Thermodynamic Properties of Heisenberg Ferrimagnetic Spin Chains Ferromagnetic-Antiferromag
CALPHAD软件介绍

Abstract
The phase-field method has become an important and extremely versatile technique for simulating microstructure evolution at the mesoscale. Thanks to the diffuse-interface approach, it allows us to study the evolution of arbitrary complex grain morphologies without any presumption on their shape or mutual distribution. It is also straightforward to account for different thermodynamic driving forces for microstructure evolution, such as bulk and interfacial energy, elastic energy and electric or magnetic energy, and the effect of different transport processes, such as mass diffusion, heat conduction and convection. The purpose of the paper is to give an introduction to the phase-field modeling technique. The concept of diffuse interfaces, the phase-field variables, the thermodynamic driving force for microstructure evolution and the kinetic phase-field equations are introduced. Furthermore, common techniques for parameter determination and numerical solution of the equations are discussed. To show the variety in phase-field models, different model formulations are exploited, depending on which is most common or most illustrative. c 2007 Elsevier Ltd. All rights reserved.
化工热力学英文词汇

thermodynamics n. 热力学 system n. 体系 thermodynamic state 热力学状态 dimension 量纲 SI= International System of Units 国际单位制 强度(热力学)变量 广度(热力学)变量celsius scale 摄氏刻度 → fahrenheit scale 华氏刻度 kelvin scale 开尔文刻度 → Rankine scale dead-weight gauge 静压、压力表 mano meter (流体)压力计 product 乘积 kinetic energy 动能 221mu E k = potential energy 势能mgz E P =conservation守恒* Terms in chapter 2sublimation curve 升华线 fusion curve 熔融线vaporization curve (蒸发)汽化线single-phase region 单相区 triple point 三相点univariant 单变量 divariant 多变量critical point 临界点 critical pressure 临界压力critical temperature 临界温度dome-shaped curve 圆拱形曲线saturated vapors at their condensation temperatures 露点的饱和蒸汽 saturated liquids at their vaporization(boiling) temperatures 泡点的饱和液体vapor pressure 蒸汽压subcooled-liquid region 过冷液体区 superheated-vapor region 过热蒸汽区partial derivative 偏导数differentiate v . 求微分,求导 differentiation n. derivate n. 求导数 derivation 求导数,求解incompressible fluid 不可压缩流体 ideal-gas理想气体simple fluid简单流体 (argon 、krypton 、xenon )virial expansion维里展开式 virial coefficients 维里系数 virial equation维里方程equation of state状态方程compressibility factor 压缩因子 RTPVZ = volume expansivity体积膨胀系数PT V V ⎪⎭⎫ ⎝⎛∂∂=1βisothermal compressibility 等温压缩系数 TP V V ⎪⎭⎫ ⎝⎛∂∂=1κ acentric factor偏心因子isothermal process 等温过程 isobaric process 等压过程 isochoric process等容过程 adiabatic process 绝热过程 polytropic process 多变过程throttling process节流过程 0=∆Htruncate equation to two terms 截断方程前二项 cubic equation of state 立方型状态方程reduced pressure 对比压力 reduced temperature 对比温度 reduced density对比密度corresponding-state parameters 对应态参数 generalized correlations 普遍化关联nonpolar非极性的 slightly polar 弱极性的 highly polar高极性的volumetric properties 容积性质 realistic 现实主义的,逼真的dashed line虚线dotted line 点线 straight line 实线Terms in chapter 3internal energy 内能 transport across kinetic energy 动能 221mu E t =potential energy 势能 m g z E p = conservation 守恒operator 算符,运算符 (such as “Δ”) system 体系 surroundings 环境 closed system 封闭体系 open system 开放体系finite change 有限的变化 infinitesimal change 无限的变化 differential change 微分(小)的变化 intensive property 强度性质 extensive property 广度性质specific or molar property 单位(比)性质或摩尔性质 property — variable — functionthermodynamics state of the system 体系热力学状态 thermodynamics properties 热力学性质 state function(s) 状态函数equilibrium 平衡 (the) phase rule 相率reversible process 可逆过程irreversible process 不可逆过程mechanically reversible 机械可逆thermostate 恒温箱constant—temperature bath 恒温浴efficiency 效率,(有效)系数enthalpy 焓heat capacity 热容constant—volume heat capacity 恒容热容constant—pressure heat capacity 恒压热容vector quantity 矢量scalar magnitude 数量,纯量continuity equation 连续方程steady state (flow process) 移去(流动过程)datum level 基准面shaft work 体积功stirring work 搅拌功work associated with moving the flow streams 流动功expansion work 膨胀功surface work 表面功electricity work 电功calorimeter 量热计(测定焓)intensive property 强度性质extensive property 广度性质shaft work 轴功enthalpy 焓entropy 熵heat-capacity 热容Gibbs energy (G) 吉布斯自由能Helmholtz energy (A) 亥姆霍茨自由能internal energy 内能system 系统,体系close system 封闭体系equilibrium state 平衡态total differential of F F的全微分exact differential expression 全微分表达式Maxwell equations 麦克斯威尔方程homogeneous fluid 均相流体residual property 剩余性质real gas 真实气体actual gasideal gas 理想气体explicit function 显函数volume explicit 体积显函数pressure explicit 压力显函数isentropic process 等熵过程reversible adiabatic process 绝热可逆过程pseudocritical parameter 虚拟临界参数path variables 过程变量state variables 状态变量constant pressure heat capacity CP 等压热容constant volume heat capacity C V 等容热容residual property 剩余性质reference state 参比态reference conditionpartial derivative 偏导数total derivative 全导数β volume expansivity 体积膨胀系数κ isothermal compressibility 等温压缩系数quality 干度fugacity 逸度fugacity coefficient 逸度系数*Terms in Chapter 4chemical potential 化学势,化学位partial property 偏性质partial molar property 偏摩尔性质ideal solution 理想溶液real solution 真实溶液excess property 超额/过量性质excess Gibbs energy 超额/过量自由焓partial excess property 偏摩尔超额/过量性质activity 活度activity coefficient 活度系数standard state 标准态property change of mixing 混合性质regular solution 正规溶液atherpical solution 无热溶液local-composition 局部组成local molar fraction 局部摩尔分数*Terms in Chapter 5First Law of thermodynamics(energy conservation law)热力学第一定律steady-state flow processes 稳定状态流动过程control volume 控制体heat Engines 热机Carnot engine 卡诺热机thermal efficiency 热效率thermodynamic efficiency 热力学效率isentropic efficiency 等熵效率ideal work and lost work 理想功和损耗功exergy 火用available Energy, availability, utilizable Energy 有效能*Terms in Chapter 6steam Power cycle 蒸汽动力循环Carnot-engine cycle 卡诺循环cycle with feed water heaters 抽气回热循环heat-power cycle 热电循环exhaust steam 乏气heat reservoir 热源working substance of the engine 工质specific steam consumption 汽耗率SSCrefrigeration Cycle 制冷循环vapor-compression cycle 蒸汽压缩(制冷)循环absorption refrigeration 吸收式制冷Carnot refrigeration 卡诺冷机reversed heat-engine cycle 逆热机循环multi-stage compression refrigeration多级压缩制冷heat pump 热泵throttling expansion process 节流膨胀过程reversible adiabatic expansion process 可逆绝热膨胀过程inversion curve and inversion point 转变曲线和转变点condenser 冷凝器expander 膨胀机compressor 压缩机evaporator 蒸发器supheater 过热器turbine 透平机boiler 锅炉pump 泵*Statements of the second lawstatement1: No apparatus can operate in such a way that its only effect (in system and surrounings) is to convert heat absorbed by a system completely into work done by the system。
超声矿物的溶解热力学模型

超声矿物的溶解热力学模型英文回答:Thermodynamic Modeling of Mineral Dissolution in Ultrasonic Environments.Ultrasonic waves, characterized by frequencies above the audible range (typically >20 kHz), possess unique properties that can influence the dissolution behavior of minerals. The application of ultrasonic irradiation to mineral dissolution processes has been shown to enhance dissolution rates and alter reaction pathways.The thermodynamic modeling of mineral dissolution under ultrasonic conditions requires consideration of the complex interplay between ultrasonic effects and the intrinsic properties of the mineral and solution. Several factors contribute to the enhanced dissolution observed in ultrasonic environments:Acoustic cavitation: Ultrasonic waves generate cavitation bubbles, which violently collapse, creating localized high-temperature and high-pressure zones. These extreme conditions facilitate mineral surface erosion and disrupt the diffusion boundary layer, enhancing mass transfer.Surface activation: Ultrasonic irradiation promotes mineral surface activation, increasing the number of active sites available for dissolution. This occurs through mechanical erosion, breaking down surface bonds and exposing fresh mineral surfaces.Hydrodynamic effects: Ultrasonic waves createturbulent flow patterns, which increase the fluid velocity and shear stress on mineral surfaces. This enhanced hydrodynamic environment promotes particle erosion and prevents the formation of stagnant zones.Temperature and pressure effects: Cavitation-induced localized heating and pressure changes can influence mineral dissolution kinetics. The elevated temperaturesincrease the dissolution rate of many minerals, while the pressure changes can affect the solubility and speciation of dissolved ions.To develop a comprehensive thermodynamic model for mineral dissolution under ultrasonic conditions, researchers must account for these factors and their combined effects. The model should incorporate the following elements:Thermodynamic database: A comprehensive thermodynamic database containing the necessary thermodynamic parameters for the mineral, solution species, and potential reaction products.Acoustic cavitation model: A model that simulates the generation and collapse of cavitation bubbles, estimating the localized temperature and pressure conditions.Surface activation model: A model that describes the surface activation process under ultrasonic irradiation, predicting the increase in active dissolution sites.Hydrodynamic model: A model that calculates the fluid velocity and shear stress on mineral surfaces, considering the ultrasonic-induced turbulence.Coupling of models: The integration of theseindividual models to simulate the coupled effects of ultrasound on mineral dissolution kinetics.By combining these elements, researchers can develop a robust thermodynamic model that accurately predicts the dissolution behavior of minerals in ultrasonic environments. Such a model would have significant applications in fields such as mineral processing, environmental remediation, and materials science.中文回答:超声条件下矿物溶解的热力学模型。
MolecularThermodynamicsPDF:分子热力学的PDF

Molecular ThermodynamicsBy John SimonMolecular Thermodynamics Details:Molecular Thermodynamics: Donald A. McQuarrie, John D. Simon ...Evolved from McQuarrie and Simon's best-selling textbook, Physical Chemistry: A Molecular Approach, this text focuses in on the thermodynamics portion of the course. Thermodynamics - Wikipedia, the free encyclopediaThermodynamics is a branch of natural science concerned with heat and its relation to energy and work. It defines macroscopic variables (such as temperature, internal energy, entropy, and pressure) that characterize materials and radiation, and explains how they are related and by what laws they ...Molecular Thermodynamics - Johns Hopkins UniversityMolecular Thermodynamics. The explosion in structural data on bio-molecules provides new opportunities to understand their function in terms of their structure. Thermochemistry - Wikipedia, the free encyclopediaThermochemistry is the study of the energy and heat associated with chemical reactions and/or physical transformations. A reaction may release or absorb energy, and a phase change may do the same, such as in melting and boiling. Thermochemistry focuses on these energy changes, particularly on ...Molecular Thermodynamics, Donald A. McQuarrie and John D. SimonMolecular Thermodynamics, by Donald McQuarrie and John Simon, published in 1999 by University Science Books.Statistical Molecular Thermodynamics | CourseraStatistical Molecular Thermodynamics is a free online class taught by Dr. Christopher J. Cramer of University of MinnesotaMolecular Thermodynamics - Donald Allan McQuarrie, John ...Evolved from McQuarrie and Simon's best-selling Physical Chemistry: A Molecular Approach, this text follows a similar path by first covering the principles of quantum mechanics before engaging those ideas in the subsequent development of thermodynamics. Although many of the chapters are similar ...Introduction to Molecular ThermodynamicsIntroduction to Molecular Thermodynamics. by Robert M. Hanson and Susan Green, St. OlafCollege.enquiries:******************.Publisher'swebsiteforthisbookMolecular Thermodynamics (Physical chemistry monograph series ...Molecular Thermodynamics (Physical chemistry monograph series) [Richard E. Dickerson] on . *FREE* shipping on qualifying offers.Molecular Thermodynamics — ITQB - Universidade Nova de LisboaMolecular Thermodynamics of Liquids and Liquid Solutions, in particular, studies of Ionic Liquids and ionic liquid-containing systems constitute the main activity.Molecular thermodynamics of fluid-phase equilibria - J. M ...Molecular Thermodynamics of Fluid-Phase Equilibria, Third Edition is a systematic, practical guide to interpreting, correlating, and predicting thermodynamic properties used in mixture-related phase-equilibrium calculations. Completely updated, this edition reflects the growing maturity of ...Molecular thermodynamics - definition of Molecular ...ther·mo·chem·is·try (thûr m-k m-str) n. The chemistry of heat and heat-associated chemical phenomena. ther mo·chem i·cal (--k l) adj. ther mo·chem ist n.Prausnitz Group Home Page - University of California, BerkeleyMolecular Thermodynamics John M. Prausnitz. Professor, University of California, Berkeley Faculty Senior Scientist, Lawrence Berkeley National LaboratorymThermoWait for more... ... The website is going through major upgrade ,, wait for the new look soon :)Molecular thermodynamics - HmolpediaAn encyclopedia of topics on the thermodynamics, chemistry, and physics of human existence.Molecular Thermodynamics of Fluid-Phase Equilibria / Edition ...The classic guide to mixtures, completely updated with new models, theories, examples, and data. Efficient separation operations and many other chemical processes depend upon a thorough understanding of the properties of gaseous and liquid mixtures. Molecular Thermodynamics of Fluid-Phase ...Molecular Thermodynamics of Fluid-Phase EquilibriaThermodynamics Home - Chemical Engineering - Thermodynamics: Molecular Thermodynamics of Fluid-Phase Equilibria by Rudiger N. Lichtenthaler, Edmundo Gomes De Azevedo, John M. Prausnitz, Edmundo G. De AzevedoMolecular thermodynamics definition of Molecular ...thermochemistry [¦th?r·m??kem·?·str?] (physical chemistry) The measurement, interpretation, and analysis of heat changes accompanying chemical reactions and changes in state.Molecular Thermodynamics by Donald A. McQuarrie — Reviews ...Molecular Thermodynamics has 7 ratings and 1 review. Jacob said: This books is incredible. It's extremely comprehensive. If you love physical chemistry a...Introduction to Molecular Thermodynamics, by Robert M. Hanson ...Introduction to Molecular Thermodynamics, by Robert M. Hanson and Susan Green , University Science Books, copyright 2008Molecular Thermodynamics of Fluid Phase Equilibria - 3rd Edition2Molecular Thermodynamics of Fluid Phase Equilibria - 3rd Edition2 - Free ebook download as PDF File (.pdf), Text file (.txt) or read book online for free.Molecular thermodynamics (Book, 1999) []Get this from a library! Molecular thermodynamics. [Donald A McQuarrie; John D Simon]Molecular Thermodynamics - Download for freeMolecular Thermodynamics download from FileSnail - 3188189138905X pdf, Thermodynamics Processes an rar, FilePost DepositFiles and RapidShare files. Molecular Thermodynamics of Fluid Phase Equilibria (Prausnitz ...Molecular Thermodynamics of Fluid Phase Equilibria (Prausnitz- 2th Edition ) - Free ebook download as Text file (.txt), PDF File (.pdf) or read book online for free. k Molecular thermodynamics (Open Library)Molecular thermodynamics by Richard Earl Dickerson, 1969,W. A. Benjamin edition, in EnglishMolecular thermodynamics - definition of Molecular ...Molecular thermodynamics. Molecular Time Scale Generalized Langevin Equation Molecular Typing by Reverse Dot-Blotting Molecular Typing Working Group Molecular Unit Cell Approximation molecular velocity Molecular vibration Molecular Vibrational SpectrometerCenter for Molecular & Engineering ThermodynamicsThe University of Delaware’s Thomas H. Epps, III, is one of 30 early-career engineers nationwide invited to attend the 2013 European Union-United States Frontiers of Engineering Symposium to be held Nov. 21-23 in Chantilly, France.ChemE | Thermodynamics and Molecular ComputationsThermodynamics and Molecular Computations. Thermodynamics governs processes as diverse as chemical production, bioreaction, creation of advanced materials, protein separation, and environmental treatment.Molecular Thermodynamics Mcquarrie : ?g Rt K Q. Molecular ...pdf document tagged with molecular thermodynamics mcquarrie : ?G RT K Q. user guide pdf categorized in molecular thermodynamics mcquarrieMolecular Thermodynamics of Fluid-Phase Equilibria by John M ...Molecular Thermodynamics of Fluid-Phase Equilibria has 7 ratings and 1 review. Theclassic guide to mixtures, completely updated with new models, theorie...。
氨水物性

28
APPENDIX A - THE IAPWS FORMULATION FOR THE THERMAL CONDUCTIVITY OF ORDINARY WATER SUBSTANCE
FOR INDUSTRIAL USE
29
APPENDIX B - THE IAPWS FORMULATION FOR THE DYNAMIC VISCOSITY OF ORDINARY WATER SUBSTANCE FOR
Unit
K bar
kJ.kg-1 kJ.kg-1.K-1 m2.s-1 kg.m-3 mW.m-1.K-1 :Pa.s mN.m-1 -
2 / 34 Properties of Working Fluids - NH3 H2O
M. CONDE ENGINEERING — 2004
2.
INTRODUCTION
22
13.
DYNAMIC VISCOSITY OF SOLUTIONS IN THE VAPOUR PHASE
25
14.
DENSITY OF SATURATED SOLUTIONS IN THE VAPOUR PHASE
26
15.
SPECIFIC THERMAL CAPACITY OF SATURATED SOLUTIONS IN THE VAPOUR PHASE
8
6.
SPECIFIC THERMAL CAPACITY OF SATURATED LIQUID SOLUTIONS AT CONSTANT PRESSURE
9
7.
THERMAL CONDUCTIVITY OF LIQUID SOLUTIONS
11
8.
热力学的本质

The Nature of Thermodynamics(热力学的本质)Thermodynamics is one of the most important areas of engineering science.It is the science used to explain how most things to work, why some things do not work the way that they were intended, and why other things just cannot possibly work at all.(热力学是工程学最重要的领域之一。它是一门科学,被用于解释大多数现象如何工作,为什么一些过程不能按指定的方式工作,为什么一些过程根本就不能工作。)It is a key part of the science engineers use to design automotive engines, heat pumps, rocker motors, power stations, gas turbines,airconditioners,superconducting transmission lines,solar heating systems,etc.(它是工程师用于设计汽车发动机、热泵、旋转式发动机、发电站、气体涡轮机、空气调节器、超导传输线、太阳能加热系统的关键知识之一。)Thermodynamics centers about the notions of energy;the idea that energy is conserved is the first law of thermodynamics. It is the starting point for the science of thermodynamics and for engineering analysis.(热力学是围绕能量的概念展开的。能量守恒的思想就是热力学第一定律。热力学第一定律是热力学和工程解析法的起点。)A second concept in thermodynamics is entropy; entropy provides a means for determining if a process is possible. Processes which produce entropy are possible; those which destroy entropy are impossible.This idea is the basis for the second law of the rmodynamics.It also provides the basis for an engineering analysis in which one calculates the maximum amount of useful power that can be obtained from a given energy source,or the minimum amount of power input required to do a certain task.(热力学中的第二个概念是熵。熵提供了确定一个过程是否可能发生的方法。熵增加的过程是可能的,熵减小的过程是不可能的。这个思想是热力学第二定律的基础,也为工程解析法提供了基础。依托工程解析法,人们可以计算出从一给定能量源中可以获得的最大有用能源的量。也可以计算出一个具体过程工作所需要的最小能量。)A clear understanding of the ideas of energy and entropy is essential for one who needs to use thermodynamics in engineering analysis.Scientists are interested in using thermodynamics to predict and relate the properties of matter,engineers are interested in using this data,together with the basic ideas of energy conservation and entropy production,to analyze the behavior of complex technological systems. There is an example of the sort of system of interestto engineers, a large central power station. In this particular plant the energy source is petroleum in one of several forms,or sometimes natural gas,and the function of the plantis to convert as much of this energyas possible to electric energy and to send this energy down the transmission line.(清晰的理解能量和熵的概念对于在工程分析中需应用热力学的人来说是至关重要的。科学家们对运用热力学预测和叙述物质的性质有着浓厚的兴趣,工程师也热衷于应用热力学,他们利用能量守恒和熵增原理去分析复杂技术系统的动态。例如,一个大型的发电站。在这个特殊的装置中,能量来源是以某种形式存在的石油,有时是天然气。装置的功能是尽可能的将这种能量转换成电能,并利用传输线将电能进行传送。) Simply expressed,the plant does this by boiling water and using the steam to turn a turbine which turns an electric generator.The simplest such power plants are able to convert only about 25 percent of the fuel energy to electric energy. But this particular plant converts approximately 40 percent it has been ingeniously designed through careful, applicator of the basic principles of thermodynamics to the hundreds of components in the system. The design engineers who made these calculations used data on the properties of steam developed by physical chemists who in turn used experimental measurements in concert with thermodynamic theory to develop the propertydata.(简单的说,该装置的作用就是使水沸腾,然后运用蒸汽推动驱动发电机的涡轮机运转。这种最简单的装置只能将25%的燃料能量转换为电能。但是独特一点的该装置能量转化率能达到40%。该装置被精心的设计,将热力学的基本定律运用到了系统中数百的部件中。设计工程师运用了蒸汽的物性数据,蒸汽热物性数据是由物理化学家通过实验测量的方法获得,他是同利用热力学理论获得的热物性数据是一致的。)Plants presently being studied could convert as much as 55 percent of the fuel energy to electric energy,if they in deed per form as predicted by thermodynamic analysis(目前正在研制的该装置其发电转换效率能达到55%,如果他们的动态能达到依据热力学分析的那样的话。)。
Stephen_William_Hawking霍金简介

Stephen William Hawking4
• During his postgraduate program, Hawking was diagnosed as having Amyotrophic Lateral Sclerosis (ALS), a rare progressive disease that handicaps movement and speech. This disease makes it necessary for Hawking to carry out the long and complex mathematical calculations that his work requires in his head. He has been able to continue his studies and to embark upon a distinguished and productive scientific career despite his illness.
Stephen William Hawking1
• whose main field of research has been the nature of space and time, including irregularities in space and time known as singularities. Hawking has also devoted much of his life to making his theories accessible to the public through lectures, books, and films. • Hawking, Stephen William (1942- ), British theoretical physicist and mathematician Hawking, Stephen William (1942- ), British theoretical physicist and mathematician whose main field of research has been the nature of space and time, including irregularities in space and time known as singularities. Hawking has also devoted much of his life to making his theories accessible to the public through lectures, books, and films.
The Thermodynamics of the Earths Atmosphere

The Thermodynamics of the Earths Atmosphere The Earth's atmosphere is a complex system that interacts with the planet's surface, oceans, and biosphere. The study of the thermodynamics of the atmosphere is essential in understanding the behavior of this system and how it affects our planet. Thermodynamics is the study of the relationships between heat, energy, and work. In the context of the Earth's atmosphere, thermodynamics helps us understand the processes that govern the movement of air, the formation of weather patterns, and the distribution of energy throughout the system.One of the key principles of thermodynamics is the conservation of energy. This principle states that energy cannot be created or destroyed; it can only be transferred or converted from one form to another. In the Earth's atmosphere, energy is transferred through a variety of processes, including radiation, conduction, and convection. Radiation is the transfer of energy through electromagnetic waves, such as those from the sun. Conduction is the transfer of energy through direct contact, such as when the ground heats the air above it. Convection is the transfer of energy through the movement of fluids, such as when warm air rises and cool air sinks.Another important principle of thermodynamics is the second law of thermodynamics, which states that the total entropy of a closed system always increases over time. Entropy is a measure of the disorder or randomness of a system. In the Earth's atmosphere, entropy increases as energy is transferred from one place to another. This means that the atmosphere tends towards a state of maximum disorder, which can lead to the formation of weather patterns and other complex phenomena.The thermodynamics of the Earth's atmosphere also plays a crucial role in the global climate system. The atmosphere acts as a greenhouse, trapping heat from the sun and regulating the temperature of the planet. This is known as the greenhouse effect, and it is essential for life on Earth. However, human activities such as the burning of fossil fuels have increased the concentration of greenhouse gases in the atmosphere, leading to an enhanced greenhouse effect and global warming. Understanding the thermodynamics ofthe atmosphere is therefore crucial in addressing the challenges of climate change and developing strategies to mitigate its impacts.From a human perspective, the thermodynamics of the Earth's atmosphere has a profound impact on our daily lives. Weather patterns such as hurricanes, tornadoes, and thunderstorms are all driven by the movement of air and the transfer of energy through the atmosphere. These phenomena can have devastating effects on communities, causing loss of life and property damage. Understanding the thermodynamics of the atmosphere can help us predict and prepare for these events, improving our ability to respond and recover from natural disasters.In conclusion, the study of the thermodynamics of the Earth's atmosphere is essential in understanding the behavior of this complex system and its impact on our planet. Through the principles of conservation of energy and the second law of thermodynamics, we can gain insights into the processes that govern the movement of air, the formation of weather patterns, and the distribution of energy throughout the system. From a human perspective, this knowledge is critical in predicting and preparing for natural disasters and addressing the challenges of climate change. As we continue to explore the mysteries of our planet's atmosphere, the principles of thermodynamics will undoubtedly play a central role in our understanding of this fascinating and complex system.。
海森堡英语介绍

海森堡英语介绍Werner Heisenberg was a pivotal figure in the development of quantum mechanics, a branch of physics that describes the physical properties of nature at the scale of atoms and subatomic particles. Born on December 5, 1901, in Würzburg, Germany, Heisenberg exhibited exceptional mathematicaltalents early in his life, which directed him towards a career in theoretical physics.Heisenberg is perhaps best known for formulating the Uncertainty Principle in 1927, a fundamental theory in quantum mechanics. This principle states that it is impossible to simultaneously know the exact position and momentum of a particle. The better we know one of these values, the less precisely we can know the other. This idea has profound implications for the nature of reality and challenges classical notions of determinism, suggesting thatat a quantum level, the universe behaves in unpredictable and probabilistic ways.In 1925, Heisenberg published his groundbreaking work titled "Quantum-theoretical Renormalization Method," which laid the foundation for matrix mechanics, the first complete formulation of quantum mechanics. Unlike previous theories, Heisenberg’s approach did not rely on the visualizable trajectories of particles. Instead, he utilized mathematical matrices to represent observable quantities, revolutionizing how physicists understand and calculate quantum phenomena.For his contributions to physics, Heisenberg was awarded the Nobel Prize in Physics in 1932. His work not only deepened the understanding of atomic structure and behavior but also triggered philosophical debates about the nature of knowledge and reality in the quantum realm.During the rise of the Nazi regime in Germany, Heisenberg grappled with the ethical implications of his work,particularly in the context of nuclear physics and its military applications. He remained a prominent figure in wartime Germany, working on nuclear research. His involvement in Germany’s atomic bomb project has sparked considerable debate regarding his intentions and the moralresponsibilities of scientists.Post-World War II, Heisenberg continued to contribute significantly to theoretical physics and also engaged in public discourse on the philosophy of science. He emphasized the importance of understanding the implications ofscientific advancements and advocated for the peaceful use of nuclear energy. Heisenberg’s legacy endures, as his insights continue to influence various fields of science and philosophy. He passed away on February 1, 1976, leaving behind a profound impact on both the understanding of quantum mechanics and the ethical considerations surroundingscientific exploration.。
物理学专业英语词汇
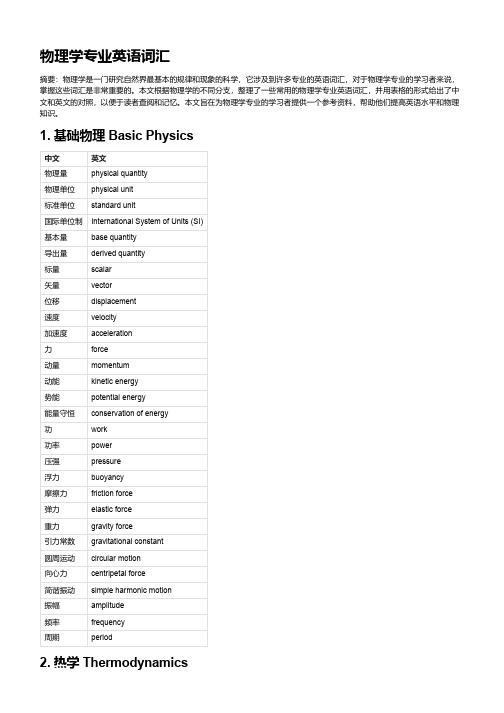
物理学专业英语词汇摘要:物理学是一门研究自然界最基本的规律和现象的科学,它涉及到许多专业的英语词汇,对于物理学专业的学习者来说,掌握这些词汇是非常重要的。
本文根据物理学的不同分支,整理了一些常用的物理学专业英语词汇,并用表格的形式给出了中文和英文的对照,以便于读者查阅和记忆。
本文旨在为物理学专业的学习者提供一个参考资料,帮助他们提高英语水平和物理知识。
1. 基础物理 Basic Physics中文英文物理量physical quantity物理单位physical unit标准单位standard unit国际单位制International System of Units (SI)基本量base quantity导出量derived quantity标量scalar矢量vector位移displacement速度velocity加速度acceleration力force动量momentum动能kinetic energy势能potential energy能量守恒conservation of energy功work功率power压强pressure浮力buoyancy摩擦力friction force弹力elastic force重力gravity force引力常数gravitational constant圆周运动circular motion向心力centripetal force简谐振动simple harmonic motion振幅amplitude频率frequency周期period2. 热学 Thermodynamics中文英文温度temperature热力学温标thermodynamic temperature scale 开尔文温标Kelvin temperature scale摄氏温标Celsius temperature scale华氏温标Fahrenheit temperature scale热平衡thermal equilibrium热力学第零定律zeroth law of thermodynamics热量heat热容量heat capacity比热容specific heat capacity理想气体定律ideal gas law普适气体常数universal gas constant3. 光学 Optics中文英文光light光源light source光线light ray光束light beam光波light wave波长wavelength频率frequency振幅amplitude相位phase干涉interference衍射diffraction偏振polarization光速speed of light折射率refractive index折射定律law of refraction反射定律law of reflection全反射total reflection透镜lens镜头lens焦距focal length焦点focus物镜objective lens可见光visible light紫外光ultraviolet light红外光infrared light4. 电学 Electricity中文英文电荷electric charge电流electric current电压electric voltage电阻electric resistance电阻率resistivity电容electric capacitance电容率permittivity5. 原子物理 Atomic Physics中文英文原子atom原子核atomic nucleus原子序数atomic number原子量atomic mass原子半径atomic radius原子轨道atomic orbit电子electron质子proton中子neutron电子云electron cloud电子壳层electron shell价电子valence electron离子ion同位素isotope同素异形体allotrope核裂变nuclear fission核聚变nuclear fusion核反应堆nuclear reactor核武器nuclear weapon6. 量子物理 Quantum Physics中文英文量子quantum量子力学quantum mechanics量子场论quantum field theory量子数quantum number量子态quantum state量子纠缠quantum entanglement量子隧穿quantum tunneling测不准原理uncertainty principle薛定谔方程Schrödinger equation海森堡矩阵力学Heisenberg matrix mechanics 7. 固体物理 Solid State Physics中文英文固体solid晶体crystal晶格lattice晶胞unit cell晶面指数Miller index点阵常数lattice constant点缺陷point defect线缺陷line defect8. 电磁学 Electromagnetism中文英文电荷electric charge电流electric current电场electric field电势electric potential电压electric voltage电阻electric resistance电阻率resistivity电容electric capacitance电容率permittivity电感electric inductance电磁感应electromagnetic induction电磁波electromagnetic wave磁场magnetic field磁通量magnetic flux磁感应强度magnetic induction intensity磁化率magnetic susceptibility磁导率magnetic permeability9. 光子学 Photonics中文英文光子photon光源light source光纤optical fiber光波导optical waveguide光谱spectrum光谱仪spectrometer激光器laser半导体激光器semiconductor laser激光二极管laser diode发光二极管light-emitting diode (LED)光探测器photodetector光电倍增管photomultiplier tube (PMT) 10. 流体力学 Fluid Mechanics中文英文流体fluid气体gas液体liquid粘性viscosity粘滞力viscous force流速flow velocity流量flow rate流线streamline管流pipe flow层流laminar flow湍流turbulent flow雷诺数Reynolds number伯努利方程Bernoulli's equation压力差pressure difference水头head水锤现象water hammer11. 波动光学 Wave Optics中文英文光波light wave波前wavefront光程差optical path difference干涉条纹interference fringe干涉仪interferometer杨氏双缝实验Young's double-slit experiment 迈克尔逊干涉仪Michelson interferometer法布里-珀罗干涉仪Fabry-Perot interferometer衍射现象diffraction phenomenon衍射级数diffraction order中文英文衍射极限diffraction limit单缝衍射single-slit diffraction双缝衍射double-slit diffraction12. 相对论 Relativity中文英文相对论relativity狭义相对论special relativity广义相对论general relativity惯性系inertial frame参考系reference frame洛伦兹变换Lorentz transformation洛伦兹收缩Lorentz contraction时间膨胀time dilation质能方程mass-energy equation光速不变原理principle of constancy of light speed 相对性原理principle of relativity引力场gravitational field引力波gravitational wave弯曲的时空curved spacetime13. 核物理 Nuclear Physics中文英文核物理nuclear physics原子核atomic nucleus核子nucleon质子proton中子neutron核力nuclear force核结合能nuclear binding energy核裂变nuclear fission核聚变nuclear fusion放射性元素radioactive element放射性衰变radioactive decay半衰期half-lifeα衰变alpha decayβ衰变beta decay。
热力学 动力学英语

热力学动力学英语Thermodynamics and Kinetics: Understanding the Fundamentals of Energy and Motion.Thermodynamics and kinetics are two branches of physics that deal with the study of energy and motion at different scales. While thermodynamics focuses on the transfer ofheat and the conversion of energy between different forms, kinetics deals with the rates of chemical reactions and the motion of particles. Together, these two branches provide a comprehensive understanding of the behavior of matter and energy in a wide range of systems.Thermodynamics is concerned with the macroscopic properties of systems, such as temperature, pressure, volume, and entropy. It describes how these properties change as energy is transferred or converted within a system. The zeroth law of thermodynamics states that if two systems are each in thermal equilibrium with a third system, then the two systems will also reach thermal equilibriumwith each other. This law establishes the concept of temperature as a measure of thermal equilibrium.The first law of thermodynamics, also known as the law of conservation of energy, states that energy cannot be created or destroyed, but can only be transformed from one form to another. This law is fundamental to all branches of physics and is expressed mathematically as delta U = Q W, where delta U represents the change in internal energy of a system, Q represents the heat transferred to the system, and W represents the work done on the system.The second law of thermodynamics states that the entropy of a closed system always increases or remains constant. Entropy is a measure of the disorder or randomness of a system. This law implies that natural processes tend to proceed in the direction of increasing entropy, or disorder. For example, when two gases are mixed together, they spontaneously diffuse throughout the container, increasing the entropy of the system.Kinetics, on the other hand, is concerned with therates of chemical reactions and the motion of particles. It studies the mechanisms and rates of chemical reactions, as well as the motion of particles in gases, liquids, and solids. Kinetics provides insights into the dynamicbehavior of matter at the atomic and molecular level.Reaction rates are described by rate laws, whichexpress the relationship between the concentration of reactants and the rate of the reaction. The rate constant, which is specific to each reaction, quantifies the rate at which the reaction proceeds under a given set of conditions. The activation energy, which represents the minimum amountof energy required to initiate a reaction, is another important concept in kinetics.Particle motion is described by the laws of motion, which govern the behavior of objects under the influence of forces. These laws, which were formulated by Isaac Newton, include the law of inertia (an object will remain at restor in uniform motion unless acted upon by an external force), the law of acceleration (the rate of change of momentum of an object is proportional to the applied forceand inversely proportional to its mass), and the law of action and reaction (for every action, there is an equal and opposite reaction).The study of thermodynamics and kinetics is crucial to understanding a wide range of phenomena in physics, chemistry, and engineering. For example, thermodynamics is essential in the design of efficient energy conversion systems, such as power plants and engines. Kinetics plays a key role in the development of catalysts and in the optimization of chemical processes. By combining the principles of thermodynamics and kinetics, scientists and engineers can gain a deeper understanding of the behavior of matter and energy and create more effective and sustainable systems.In conclusion, thermodynamics and kinetics are two interrelated branches of physics that provide a fundamental understanding of energy and motion. Thermodynamics focuses on the macroscopic properties of systems and the transfer of energy between different forms, while kinetics deals with the rates of chemical reactions and the motion ofparticles. By studying these branches together, we can gain a more comprehensive understanding of the behavior of matter and energy and apply this knowledge to create innovative and sustainable systems.。
热力学计算英文

热力学计算英文Thermodynamics, a branch of physics dealing with the relationship between heat and other forms of energy, such as work, plays a pivotal role in various engineering and scientific fields. Understanding and mastering thermodynamic calculations are crucial for engineers, physicists, and chemists alike, as they provide insights into energy conversions, efficiencies, and equilibria in systems.**Basics of Thermodynamic Calculations**Thermodynamic calculations often involve the laws of thermodynamics, which outline the fundamental principles governing energy transfers and conversions. The Zeroth Law establishes the concept of thermodynamic equilibrium, stating that if two systems are each in thermal equilibrium with a third system, then the two systems will reach thermal equilibrium with each other. The First Law, known as the Law of Conservation of Energy, states that energy cannot be created or destroyed, but only transformed from one form to another. The Second Law, often referred to as the Law of Entropy, outlines the directionality of naturalprocesses, stating that the entropy of an isolated system always increases or remains constant.**Applications of Thermodynamic Calculations**Thermodynamic calculations find widespread applications in various industries and research fields. In the automotive industry, for instance, thermodynamic calculations are employed to analyze engine efficiency,fuel consumption, and exhaust emissions. In the chemical industry, they play a vital role in reaction optimization, predicting equilibrium constants, and understanding reaction mechanisms. Furthermore, thermodynamiccalculations are also crucial in energy conversion systems, such as solar cells and fuel cells, where they help in optimizing efficiency and understanding energy losses.**Key Concepts in Thermodynamic Calculations**Several key concepts underlie thermodynamic calculations, including entropy, enthalpy, and Gibbs free energy. Entropy is a measure of disorder or randomness in a system, and it plays a crucial role in determining the directionality of natural processes. Enthalpy represents the total heat content of a system, accounting for bothinternal energy and the work done by or on the system.Gibbs free energy is a thermodynamic potential thatindicates whether a process will occur spontaneously or not, taking into account both entropy and enthalpy changes.**Advanced Thermodynamic Calculations**Advanced thermodynamic calculations involve more complex concepts and equations, such as those dealing with multi-component systems, non-ideal gases, and phase transitions. These calculations require a deepunderstanding of thermodynamics and a proficiency in mathematical techniques such as partial derivatives and integration. By mastering these advanced calculations, engineers and scientists can gain a deeper understanding of complex systems and develop more efficient and sustainable technologies.**Conclusion**Thermodynamic calculations are essential for understanding and optimizing energy conversions, efficiencies, and equilibria in various systems. By mastering the basics and exploring advanced concepts, engineers and scientists can make significant contributionsto fields ranging from automotive design to renewableenergy research. As the world continues to grapple with energy and environmental challenges, thermodynamic calculations will play a pivotal role in developing sustainable and efficient solutions.**热力学计算的深入探索**热力学是物理学的一个分支,研究热量与其他能量形式(如功)之间的关系,在工程和科学领域的各个分支中发挥着至关重要的作用。
工程热力学英文版1

CHAPTER1INTRODUCTION1.1What is thermodynamics?Thermodynamics is the science which has evolved from the original investiga-tions in the19th century into the nature of“heat.”At the time,the leading theory of heat was that it was a type offluid,which couldflow from a hot body to a colder one when they were brought into contact.We now know that what was then called“heat”is not afluid,but is actually a form of energy–it is the energy associated with the continual,random motion of the atoms which compose macroscopic matter,which we can’t see directly.This type of energy,which we will call thermal energy,can be converted (at least in part)to other forms which we can perceive directly(for example, kinetic,gravitational,or electrical energy),and which can be used to do useful things such as propel an automobile or a747.The principles of thermodynamics govern the conversion of thermal energy to other,more useful forms.For example,an automobile engine can be though of as a device whichfirst converts chemical energy stored in fuel and oxygen molecules into thermal en-ergy by combustion,and then extracts part of that thermal energy to perform the work necessary to propel the car forward,overcoming friction.Thermody-namics is critical to all steps in this process(including determining the level of pollutants emitted),and a careful thermodynamic analysis is required for the design of fuel-efficient,low-polluting automobile engines.In general,thermody-namics plays a vital role in the design of any engine or power-generating plant, and therefore a good grounding in thermodynamics is required for much work in engineering.If thermodynamics only governed the behavior of engines,it would probably be the most economically important of all sciences,but it is much more than that.Since the chemical and physical state of matter depends strongly on how much thermal energy it contains,thermodynamic principles play a central role in any description of the properties of matter.For example,thermodynamics allows us to understand why matter appears in different phases(solid,liquid, or gaseous),and under what conditions one phase will transform to another.1CHAPTER1.INTRODUCTION2The composition of a chemically-reacting mixture which is given enough time to come to“equilibrium”is also fully determined by thermodynamic principles (even though thermodynamics alone can’t tell us how fast it will get there).For these reasons,thermodynamics lies at the heart of materials science,chemistry, and biology.Thermodynamics in its original form(now known as classical thermodynam-ics)is a theory which is based on a set of postulates about how macroscopic matter behaves.This theory was developed in the19th century,before the atomic nature of matter was accepted,and it makes no reference to atoms.The postulates(the most important of which are energy conservation and the impos-sibility of complete conversion of heat to useful work)can’t be derived within the context of classical,macroscopic physics,but if one accepts them,a very powerful theory results,with predictions fully in agreement with experiment.When at the end of the19th century itfinally became clear that matter was composed of atoms,the physicist Ludwig Boltzmann showed that the postu-lates of classical thermodynamics emerged naturally from consideration of the microscopic atomic motion.The key was to give up trying to track the atoms in-dividually and instead take a statistical,probabilistic approach,averaging over the behavior of a large number of atoms.Thus,the very successful postulates of classical thermodynamics were given afirm physical foundation.The science of statistical mechanics begun by Boltzmann encompasses everything in classical thermodynamics,but can do more also.When combined with quantum me-chanics in the20th century,it became possible to explain essentially all observed properties of macroscopic matter in terms of atomic-level physics,including es-oteric states of matter found in neutron stars,superfluids,superconductors,etc. Statistical physics is also currently making important contributions in biology, for example helping to unravel some of the complexities of how proteins fold.Even though statistical mechanics(or statistical thermodynamics)is in a sense“more fundamental”than classical thermodynamics,to analyze practical problems we usually take the macroscopic approach.For example,to carry out a thermodynamic analysis of an aircraft engine,its more convenient to think of the gas passing through the engine as a continuumfluid with some specified properties rather than to consider it to be a collection of molecules.But we do use statistical thermodynamics even here to calculate what the appropriate property values(such as the heat capacity)of the gas should be.CHAPTER1.INTRODUCTION3 1.2Energy and EntropyThe two central concepts of thermodynamics are energy and entropy.Most other concepts we use in thermodynamics,for example temperature and pres-sure,may actually be defined in terms of energy and entropy.Both energy and entropy are properties of physical systems,but they have very different characteristics.Energy is conserved:it can neither be produced nor destroyed, although it is possible to change its form or move it around.Entropy has a different character:it can’t be destroyed,but it’s easy to produce more entropy (and almost everything that happens actually does).Like energy,entropy too can appear in different forms and be moved around.A clear understanding of these two properties and the transformations they undergo in physical processes is the key to mastering thermodynamics and learn-ing to use it confidently to solve practical problems.Much of this book is focused on developing a clear picture of energy and entropy,explaining their origins in the microscopic behavior of matter,and developing effective methods to analyze complicated practical processes1by carefully tracking what happens to energy and entropy.1.3Some TerminologyMostfields have their own specialized terminology,and thermodynamics is cer-tainly no exception.A few important terms are introduced here,so we can begin using them in the next chapter.1.3.1System and EnvironmentIn thermodynamics,like in most other areas of physics,we focus attention on only a small part of the world at a time.We call whatever object(s)or region(s) of space we are studying the system.Everything else surrounding the system (in principle including the entire universe)is the environment.The boundary between the system and the environment is,logically,the system boundary. The starting point of any thermodynamic analysis is a careful definition of the system.EnvironmentSystemBoundarySystemCHAPTER 1.INTRODUCTION4Figure 1.1:Control masses and control volumes.1.3.2Open,closed,and isolated systemsAny system can be classified as one of three types:open,closed,or isolated.They are defined as follows:open system:Both energy and matter can be exchanged with the environ-ment.Example:an open cup of coffee.closed system:energy,but not matter,can be exchanged with the environ-ment.Examples:a tightly capped cup of coffee.isolated system:Neither energy nor matter can be exchanged with the envi-ronment –in fact,no interactions with the environment are possible at all.Example (approximate):coffee in a closed,well-insulated thermos bottle.Note that no system can truly be isolated from the environment,since no thermal insulation is perfect and there are always physical phenomena which can’t be perfectly excluded (gravitational fields,cosmic rays,neutrinos,etc.).But good approximations of isolated systems can be constructed.In any case,isolated systems are a useful conceptual device,since the energy and mass con-tained inside them stay constant.1.3.3Control masses and control volumesAnother way to classify systems is as either a control mass or a control volume .This terminology is particularly common in engineering thermodynamics.A control mass is a system which is defined to consist of a specified piece or pieces of matter.By definition,no matter can enter or leave a control mass.If the matter of the control mass is moving,then the system boundary moves with it to keep it inside (and matter in the environment outside).A control volume is a system which is defined to be a particular region of space.Matter and energy may freely enter or leave a control volume,and thus it is an open system.CHAPTER1.INTRODUCTION5 1.4A Note on UnitsIn this book,the SI system of units will be used exclusively.If you grew up anywhere but the United States,you are undoubtedly very familiar with this system.Even if you grew up in the US,you have undoubtedly used the SI system in your courses in physics and chemistry,and probably in many of your courses in engineering.One reason the SI system is convenient is its simplicity.Energy,no matter what its form,is measured in Joules(1J=1kg-m2/s2).In some other systems, different units are used for thermal and mechanical energy:in the English sys-tem a BTU(“British Thermal Unit”)is the unit of thermal energy and a ft-lbf is the unit of mechanical energy.In the cgs system,thermal energy is measured in calories,all other energy in ergs.The reason for this is that these units were chosen before it was understood that thermal energy was like mechanical energy, only on a much smaller scale.2Another advantage of SI is that the unit of force is indentical to the unit of(mass x acceleration).This is only an obvious choice if one knows about Newton’s second law,and allows it to be written asF=m a.(1.1)In the SI system,force is measured in kg-m/s2,a unit derived from the3primary SI quantities for mass,length,and time(kg,m,s),but given the shorthand name of a“Newton.”The name itself reveals the basis for this choice of force units.The units of the English system werefixed long before Newton appeared on the scene(and indeed were the units Newton himself would have used).The unit of force is the“pound force”(lbf),the unit of mass is the“pound mass”(lbm)and of course acceleration is measured in ft/s2.So Newton’s second law must include a dimensional constant which converts from Ma units(lbm ft/s2) to force units(lbf).It is usually written1F=2Mixed unit systems are sometimes used too.American power plant engineers speak of the “heat rate”of a power plant,which is defined as the thermal energy which must be absorbed from the furnace to produce a unit of electrical energy.The heat rate is usually expressed in BTU/kw-hr.CHAPTER1.INTRODUCTION6In practice,the units in the English system are now defined in terms of their SI equivalents(e.g.one foot is defined as a certain fraction of a meter,and one lbf is defined in terms of a Newton.)If given data in Engineering units,it is often easiest to simply convert to SI,solve the problem,and then if necessary convert the answer back at the end.For this reason,we will implicitly assume SI units in this book,and will not include the g c factor in Newton’s2nd law.。
Thermodynamics培训课件

指系统从某一初始状态出发,经历一系列变 化后又回到初始状态的过程。
03
第一定律
定义和内容
定义
第一定律又称为热力学第一定律,是指能量守恒定律在系统 和环境间的应用。
内容
能量不能被创造或消失,只能从一种形式转化为另一种形式 ,系统的总能量保持不变。
热力学能和焓
热力学能
热力学能是指系统在一定压力和温度下的总能量,包括内能和动能。
Understand energy conversion: 了解能量 转换过程
Understand the design of systems: 了 解系统设计中的热力学 应用
Understand the behavior of the environment: 了解环 境中热力学avior of matter in the universe: 了解宇宙 中物质在热力学下的行 为
状态变量
描述系统状态的物理量或化学量,称为状态变量 。
基本状态变量
温度、压力、体积、物质的量等。
过程与循环
过程
热过程
指系统从某一初始状态出发,通过输入或输 出物质或能量,变为另一状态的过程。
指只涉及温度变化的过程,热过程中不发生 化学反应和相变。
平衡过程
循环过程
指系统中所有位置上的物理量和化学量都保 持恒定的过程。
3
该定律有几种表述方式,如克劳修斯表述、开 尔文表述、熵增加原理等。
熵和熵增加原理
熵是描述系统“不可用”能量的一个物理量,可以表示能量的“混乱度”或“无 序度”。
熵增加原理是指,封闭系统中的熵永不减少,只能增加或保持不变。
热力学第二定律指出,在一个封闭系统中,过程总是朝着熵增加的方向进行,即 系统的熵永不减少。
热力学相关单词

thermodynamics n. 热力学 system n. 体系 thermodynamic state 热力学状态 dimension 量纲 SI= International System of Units 国际单位制 intensive thermodynamic variable 强度(热力学)变量 extensive thermodynamic variable 广度(热力学)变量celsius scale 摄氏刻度 → fahrenheit scale 华氏刻度 kelvin scale 开尔文刻度 → Rankine scale dead-weight gauge 静压、压力表 mano meter (流体)压力计 product 乘积 kinetic energy 动能 221mu E k = potential energy 势能mgz E P =conservation守恒* Terms in chapter 2sublimation curve 升华线 fusion curve 熔融线vaporization curve (蒸发)汽化线single-phase region 单相区 triple point 三相点univariant 单变量 divariant 多变量critical point 临界点 critical pressure 临界压力critical temperature 临界温度dome-shaped curve 圆拱形曲线saturated vapors at their condensation temperatures 露点的饱和蒸汽 saturated liquids at their vaporization(boiling) temperatures 泡点的饱和液体vapor pressure 蒸汽压subcooled-liquid region 过冷液体区 superheated-vapor region 过热蒸汽区partial derivative 偏导数differentiate v. 求微分,求导 differentiation n. derivate n. 求导数 derivation 求导数,求解incompressible fluid 不可压缩流体 ideal-gas理想气体simple fluid简单流体 (argon 、krypton 、xenon )virial expansion维里展开式 virial coefficients 维里系数 virial equation维里方程equation of state状态方程compressibility factor 压缩因子 RT PVZ = volume expansivity体积膨胀系数PT V V ⎪⎭⎫ ⎝⎛∂∂=1βisothermal compressibility 等温压缩系数 TP V V ⎪⎭⎫ ⎝⎛∂∂=1κ acentric factor偏心因子isothermal process等温过程isobaric process 等压过程 isochoric process 等容过程 adiabatic process 绝热过程 polytropic process多变过程throttling process节流过程 0=∆Htruncate equation to two terms 截断方程前二项 cubic equation of state 立方型状态方程reduced pressure 对比压力 reduced temperature 对比温度 reduced density对比密度corresponding-state parameters 对应态参数 generalized correlations 普遍化关联nonpolar非极性的 slightly polar 弱极性的 highly polar高极性的volumetric properties 容积性质 realistic 现实主义的,逼真的dashed line虚线dotted line 点线straight line 实线Terms in chapter 3internal energy 内能 transport across kinetic energy 动能 221mu E t =potential energy 势能 mgz E p = conservation 守恒operator 算符,运算符 (such as “Δ”) system 体系 surroundings 环境 closed system 封闭体系 open system 开放体系finite change 有限的变化 infinitesimal change 无限的变化 differential change 微分(小)的变化 intensive property 强度性质 extensive property 广度性质specific or molar property 单位(比)性质或摩尔性质 property — variable — functionthermodynamics state of the system 体系热力学状态 thermodynamics properties 热力学性质 state function(s) 状态函数equilibrium 平衡 (the) phase rule 相率reversible process 可逆过程irreversible process 不可逆过程mechanically reversible 机械可逆thermostate 恒温箱constant—temperature bath 恒温浴efficiency 效率,(有效)系数enthalpy 焓heat capacity 热容constant—volume heat capacity 恒容热容constant—pressure heat capacity 恒压热容vector quantity 矢量scalar magnitude 数量,纯量continuity equation 连续方程steady state (flow process) 移去(流动过程)datum level 基准面shaft work 体积功stirring work 搅拌功work associated with moving the flow streams 流动功expansion work 膨胀功surface work 表面功electricity work 电功calorimeter 量热计(测定焓)intensive property 强度性质extensive property 广度性质shaft work 轴功enthalpy 焓entropy 熵heat-capacity 热容Gibbs energy (G) 吉布斯自由能Helmholtz energy (A) 亥姆霍茨自由能internal energy 内能system 系统,体系close system 封闭体系equilibrium state 平衡态total differential of F F的全微分exact differential expression 全微分表达式Maxwell equations 麦克斯威尔方程homogeneous fluid 均相流体residual property 剩余性质real gas 真实气体actual gasideal gas 理想气体explicit function 显函数volume explicit 体积显函数pressure explicit 压力显函数isentropic process 等熵过程reversible adiabatic process 绝热可逆过程pseudocritical parameter 虚拟临界参数path variables 过程变量state variables 状态变量等压热容constant pressure heat capacity Cconstant volume heat capacity C V 等容热容residual property 剩余性质reference state 参比态reference conditionpartial derivative 偏导数total derivative 全导数β volume expansivity 体积膨胀系数κ isothermal compressibility 等温压缩系数quality 干度fugacity 逸度fugacity coefficient 逸度系数*Terms in Chapter 4chemical potential 化学势,化学位partial property 偏性质partial molar property 偏摩尔性质ideal solution 理想溶液real solution 真实溶液excess property 超额/过量性质excess Gibbs energy 超额/过量自由焓partial excess property 偏摩尔超额/过量性质activity 活度activity coefficient 活度系数standard state 标准态property change of mixing 混合性质regular solution 正规溶液atherpical solution 无热溶液local-composition 局部组成local molar fraction 局部摩尔分数*Terms in Chapter 5First Law of thermodynamics(energy conservation law)热力学第一定律steady-state flow processes 稳定状态流动过程control volume 控制体heat Engines 热机Carnot engine 卡诺热机thermal efficiency 热效率thermodynamic efficiency 热力学效率isentropic efficiency 等熵效率ideal work and lost work 理想功和损耗功exergy 火用available Energy, availability, utilizable Energy 有效能*Terms in Chapter 6steam Power cycle 蒸汽动力循环Carnot-engine cycle 卡诺循环cycle with feed water heaters 抽气回热循环heat-power cycle 热电循环exhaust steam 乏气heat reservoir 热源working substance of the engine 工质specific steam consumption 汽耗率SSCrefrigeration Cycle 制冷循环vapor-compression cycle 蒸汽压缩(制冷)循环absorption refrigeration 吸收式制冷Carnot refrigeration 卡诺冷机reversed heat-engine cycle 逆热机循环multi-stage compression refrigeration多级压缩制冷heat pump 热泵throttling expansion process 节流膨胀过程reversible adiabatic expansion process 可逆绝热膨胀过程inversion curve and inversion point 转变曲线和转变点condenser 冷凝器expander 膨胀机compressor 压缩机evaporator 蒸发器supheater 过热器turbine 透平机boiler 锅炉pump 泵*Statements of the second lawstatement1: No apparatus can operate in such a way that its only effect (in system and surrounings) is to convert heat absorbed by a system completely into work done by the system。
The thermodynamic properties of gases

The thermodynamic properties of gasesGas is one of the fundamental states of matter, along with solid and liquid. However, unlike solids and liquids, which have clear boundaries and take up specific volumes, gases expand and occupy all the available space within a container. Gases are also characterized by their low density and highly compressible nature. Understanding the thermodynamic properties of gases is essential for many areas of science and engineering, including chemistry, physics, and environmental science.One of the key thermodynamic properties of gases is pressure. Pressure is defined as the force exerted per unit area, and it is measured in Pascals or atmospheres. In a gas, pressure is caused by the collisions of gas molecules with the walls of the container. The more collisions that occur per unit time, the higher the pressure of the gas. This relationship is encapsulated in the ideal gas law, which states that the pressure of a gas is directly proportional to the number of gas molecules, the temperature of the gas, and the volume of the container.Another important thermodynamic property of gases is temperature. Temperature is a measure of the average kinetic energy of gas molecules. In other words, the higher the temperature of a gas, the faster its molecules are moving. This is why gases tend to expand when heated and contract when cooled. Temperature is measured in degrees Kelvin (K) or Celsius (C). In scientific contexts, Kelvin is the preferred unit of measurement because it is an absolute scale that does not have negative values.The volume of a gas is another important thermodynamic property. Volume is a measure of the amount of space that a gas occupies within a container. The volume of a gas is determined by the size and shape of the container, as well as the number and behavior of the gas molecules. According to the ideal gas law, the volume of a gas is directly proportional to the number of gas molecules and the temperature of the gas, and inversely proportional to the pressure of the gas.The thermodynamic properties of gases are closely related to each other. For example, pressure, volume, and temperature form a trinity of interdependent variablesthat are described by the ideal gas law. By manipulating one of these variables, scientists and engineers can affect the others. For example, if you decrease the volume of a gas while keeping its temperature constant, its pressure will increase. Conversely, if you increase the temperature of a gas while keeping its volume constant, its pressure will also increase.The behavior of real gases may differ somewhat from that of ideal gases. Real gases can be subject to intermolecular forces such as Van der Waals forces, which can cause the gas molecules to attract or repel each other. Real gases can also experience phase changes, like condensation or liquefaction. However, most gases behave in a somewhat ideal manner under normal conditions, and the ideal gas law is a good approximation for many real-world problems.In conclusion, the thermodynamic properties of gases are fundamental to our understanding of the physical world. Pressure, temperature, and volume are the key variables that describe the behavior of gases, and they are intrinsically linked to each other. By manipulating these variables, scientists and engineers can achieve a wide range of practical goals, from producing electricity to purifying gas mixtures. Our knowledge of gas thermodynamics continues to expand as new technologies and research methods become available.。
ThermodynamicPro...

MW = 120.02
R = 8.314471 J/mole • K
Properties calculated in SI units from the equation and
constants listed above can be converted to I/P units
using the conversion factors shown below. Please note
that in converting enthalpy and entropy from SI to I/P
units, a change in reference states must be included (from H = 200 and S = 1 at 0°C for SI units to H = 0 and S = 0 at –40°C for I/P units). In the conversion equation below, H (ref) and S (ref) are the saturated liquid enthalpy and entropy at –40°C. For HFC-125, H (ref) = 152.1 kJ/kg and S (ref) = 0.8120 kJ/kg • K.
Thermodynamic Properties of HFC-125 Refrigerant (pentafluoroethane)
SI Units
New tables of the thermodynamic properties of HFC-125 have been developed and are presented here. These tables are based on experimental data from the database at the National Institute of Standards and Technology (NIST). Equations have been developed, based on the Modified Benedict-Webb-Rubin (MBWR) equation of state, which represent the data with accuracy and consistency throughout the entire range of temperature, pressure, and density.
The Investigation of Thermodynamic Properties

The Investigation of ThermodynamicProperties热力学属性的探究热力学是研究物质热力学性质和计算其物理状态的分支学科,其中包括物质的能量、热量和运动等方面的研究。
热力学属性是热力学研究中的一个重要方面,它包括热力学函数、热力学过程和热力学平衡等内容。
因此,研究热力学属性对于揭示物质的物理本质、开发新材料和优化工业生产具有重要的意义。
本文将从热力学函数、热力学过程和热力学平衡三个方面探究热力学属性的相关知识。
热力学函数热力学函数是用于描述系统的热力学状态和性质的函数。
常见的热力学函数包括内能、焓、自由能和熵等。
其中,内能和焓是系统的基本状态函数,自由能和熵是系统的热力学势函数。
内能是系统的总能量,包括系统分子和原子内部运动的动能和势能以及与周围环境之间的相互作用所带来的能量。
焓则是内能和压力乘积的和,它表示系统对外界强制作用下的响应程度。
自由能是系统的最小能量,也是系统稳定性及化学反应平衡的指标。
熵则是描述系统无序度的物理量,它是一个基本的热力学函数。
热力学过程热力学过程是系统在热力学变化过程中的一些基本规律和特征,包括热力学系统的简单过程、循环过程、空气循环过程等。
在这些过程中,物质一般会发生温度、压力、体积和热量等方面的变化。
热力学内部系统的过程分为等压过程、等容过程、等温过程和绝热过程。
等压过程指的是在恒压条件下发生的过程,等容过程指的是在恒容条件下的过程,等温过程指的是在恒温条件下的过程,绝热过程则没有热传导。
通过研究这些过程,可以更好地了解物质的基本性质和特征。
热力学平衡热力学平衡是指热力学系统中物质和能量的分布趋势达到稳定,且系统中各个部分的宏观性质不随时间而变化的状态。
热力学平衡可以分为机械平衡、热平衡和化学平衡等,其中机械平衡是指系统中各个部分在压力等相同的条件下达到的平衡状态;热平衡是指系统中各个部分达到温度相同的平衡状态;化学平衡则是指系统化学反应达到平衡状态时的状态。
Thermophysical Properties of Energy Materials

Thermophysical Properties of EnergyMaterials热物性质是评估能源材料的一个重要参数。
它们反映了材料在热力学条件下的性能,如热传导,热胀缩,比热和密度。
这些参数决定了材料在能源系统中的使用效果。
因此,了解热物性质是开发新的能源材料和优化现有能源系统的必要条件。
热物性质的测量通常涉及复杂的试验和计算,需要使用高级的实验设备和计算模型。
最常用的测量技术包括热传导测量,热膨胀测量,比热测量和密度测量。
热传导是一个材料的热导率,它是材料导热能力的度量。
它涉及能量从一个点传输到另一个点的能力。
热导率的实验测量涉及Searle法,Lee-Redwood法,Lee-Kesler法等。
这些方法可用于测定相对误差小于2%的热导率。
热膨胀是材料的尺寸随温度变化的度量。
它是材料在受热后膨胀或收缩的程度。
热膨胀通常由材料的线膨胀系数或体膨胀系数表示。
这些系数的测量可以使用奥托温度计,激光干涉仪或静态法进行。
比热是材料吸收或释放热量的能力。
它是材料每单位质量所需的能量来提高其温度的度量。
比热的测量通常使用恒压比热容和恒容比热容。
测量恒压比热容的常用方法包括Kapitza法和Dulong-Petit法。
测量恒容比热容的方法包括交流热量法和等温热量法。
密度是材料的质量和体积之比。
它是评估材料性能的重要参数。
测量方法包括放射性计数法,气相位移定量法和浮动法等。
在能源领域,热物性质的测量扮演着重要的角色。
例如,热传导率决定了热交换器的性能,热膨胀决定了材料的热稳定性,比热决定了太阳能热发电系统的效率。
因此,研究热物性质特别是能源材料的热物性质是推动能源系统创新和效率提高的关键。
近年来,随着能源领域的迅速发展,热物性质的研究和测量也得到了快速进展。
例如,使用纳米热传感器可以进行高分辨率的热传导测量。
通过使用高性能计算技术,可以更准确地模拟材料的热物性质。
此外,新方法和新设备也被开发用于测量高温和腐蚀环境下的热物性质。
The Thermodynamics of the XXZ Heisenberg Chain with Impurities

Boyu Houa , Kangjie Shia,b , Ruihong Yuea,c , Shaoyou Zhaoa
a
Institute of modern physics, Northwest university,P.O.Box 105, Xi’an 710069, P.R. China
3η ) 2
+2
i cosh η , sinh(3η )
Ei = −
i sinh(2rck + 2si η + η ) , r =±1 k =a,b 2 sinh(rck + si η ) sinh(rck + si η + η )
M 2i sinh(2η ) i cosh(2η ) i sinh(3η ) − 2N − , sinh η sinh(2η ) sinh(2η ) j =1 cosh(2vj ) − cosh(2η )
M 2i sinh η i cosh η − , sinh η j =1 cosh(2vj ) − cosh η
E∞ = −N
(3)
where si is the arbitrary spin of impurities; to spin-1 chain case, we have Eb = − 2 sinh(ξ + i sinh(2η ) ) sinh(ξ + − +η 2
arXiv:cond-mat/9910386v1 25 Oct 1999
b
CCAST (World Laboratory), P.O. Box 8730, Beijing 100080, China
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :c o n d -m a t /9710291v 2 [c o n d -m a t .s t a t -m e c h ] 28 O c t 1997Thermodynamic Properties of Heisenberg Ferrimagnetic Spin Chains:Ferromagnetic-Antiferromagnetic CrossoverShoji YamamotoDepartment of Physics,Faculty of Science,Okayama University,Tsushima,Okayama 700,JapanTakahiro FukuiInstitute of Advanced Energy,Kyoto University,Uji,Kyoto 611,Japan(Received )We study thermodynamic properties of the one-dimensional Heisenberg ferrimagnet with antifer-romagnetically exchange-coupled two kinds of spins 1and 1/2.The specific heat and the magnetic susceptibility are calculated employing a modified spin-wave theory as well as a quantum Monte Carlo method.The specific heat is in proportion to T 1/2at low enough temperatures but shows a Schottky-like peak at mid temperatures.The susceptibility diverges as T −2.We reveal that at low temperatures the model is regarded as a ferromagnet,while at mid temperatures it behaves like a gapped antiferromagnet.PACS numbers:75.10.Jm,65.50.+m,75.40.Mg,75.30.DsA great progress has been made in studying the qual-itative difference [1]between the integer-spin and the half-odd-integer-spin Heisenberg antiferromagnets.Re-cently there has appeared brand-new attempts to ex-plore the quantum behavior of mixed-spin chains with two kinds of spins.Low-energy properties of various mixed-spin chains with singlet ground states were ana-lyzed [2]via the nonlinear-σ-model technique with par-ticular emphasis on the competition between the mas-sive and the massless phases.Mixed-spin chains with magnetic ground states serve us with another topic and have in fact attracted much current interests [3–8].Since we expect a gapless excitation from the ferrimagnetic ground state,we there take little interest in the most naive problem whether the spectrum is gapped or gap-less.Performing a numerical investigation by the use of conformal invariance,Alcaraz and Malvezzi [3]indeed predicted the appearance of quadratic dispersion rela-tions for the mixed-spin Heisenberg ferrimagnets,which is consistent with a spin-wave calculation [4,5].Actually the quadratic dispersion has explicitly been visualized employing a quantum Monte Carlo (QMC)technique [6]and an exact-diagonalization method [8].Thus we may expect quantum ferrimagnets to behave like ferromagnets at low temperatures.However,several authors [4,5]have recently reported that quantum ferrimagnets have a non-trivial excitation branch gapped from the ground states as well as a gapless one,which stimulates us to investi-gate their thermodynamic properties.We show in this article that the two distinct low-lying excitations result in a novel temperature dependence of the thermal quanti-ties displaying both ferromagnetic and antiferromagnetic aspects .The present study sounds more fascinating con-sidering that all the mixed-spin-chain compounds syn-thesized so far exhibit ferrimagnetic ground states [9].We consider alternatively aligned two kinds of spins S and s on a ring with antiferromagnetic exchange cou-pling between nearest neighbors,which are described by the HamiltonianH =JN j =1(S j ·s j +s j ·S j +1)−gµB HM ,(1)where M ≡S z +s z ≡ Nj =1(S zj +s z j )is the total mag-netization,N the number of the unit cells,µB the Bohrmagneton,and we have set the g factors of the spins S and s equal to g .For the sake of argument,we as-sume throughout the manuscript that S >s .Apply-ing the Lieb-Mattis theorem [10]to the Hamiltonian (1)with no external field,we find (S −s )N -fold degenerate ground states.Therefore the model exhibits ferrimag-netism instead of antiferromagnetism.The gapless and the gapped excitations,respectively,lie in the subspaces of M <(S −s )N and M >(S −s )N and thus may be regarded as ferromagnetic and antiferromagnetic.In the case of (S,s )=(1,1/2),the gap to the antiferromagnetic branch was numerically estimated to be 1.759J [5,8].Now we present QMC calculation of the thermal quan-tities at zero field.The recent field-theoretical argument [3]and density-matrix renormalization-group (DMRG)study [4]both suggest that the low-temperature proper-ties of the model are qualitatively the same regardless of the values of S and s as long as they differ from each other.That is why we restrict our numerical investiga-tion to the case of (S,s )=(1,1/2).We employ the QMC method based on the Suzuki-Trotter decomposition [11]of checkerboard type [12]and its numerical procedure has been detailed elsewhere [13].We mainly calculate the N =32chain,which is long enough to discuss the bulk properties.Since the correlation length of the sys-tem is smaller than the length of the unit cell [4,5],the thermal quantities show no significant size dependence.We show in Fig.1temperature dependences of the specific heat C (a)and the magnetic susceptibility χ(b).Although the overall temperaturedependencesofthe re-centDMRGfindings[4]are similar to ones ofourQMCresults,the twocalculationsarenot in quantitative agree-ment with each other.We have confirmed that quantumtransfer-matrix[14]calculation for short chains preciselyreproduce the present QMCfindings except for very lowtemperatures,where overshort chains may pretend to begapped.Furthermore high-temperature series-expansioncalculation helps us to verify our numerical treatment.Within the up-to-t−3approximation,the specific heatand the magnetic susceptibility are expanded asCNg2µ2B=113t-2+112,E g≡E0+E1is the ground state en-ergy with E0=−2JSsNJ and E1= k[ωk−J(S+s)],and a is the lattice spacing.α†kandβ†kare the creationoperators of the ferromagnetic and the antiferromagneticspin waves with momentum k.The spin-S ferromagneticHeisenberg chain exhibits the spin-wave excitations witha quadratic dispersionωk=2JS[1−cos(ak)].Thus,only in the S=2s cases,the ferromagnetic branch of thespin-(S,s)ferrimagnets show exactly the same dispersionas the spin-s ferromagnets exhibit at small momenta inthe unit of the unit-cell length being unity.Hence weexpect the present model to behave like the spin-1/2fer-romagnet at low temperatures.The precise low-temperature behavior of the spin-1/2ferromagnet has been revealed by Takahashi and Yamada[15].Numerically solving the thermodynamic Bethe-ansatz integral equations,they succeeded in expandingthe thermal quantities by powers of t1/2asC2−2.00t+3.5t3Ng2µ2B=0.04167t-2+0.145t-32),(5)which are also plotted in Fig. 1.Although theQMC calculation can not reach low enough tempera-tures,yet ourfindings allow us to conclude that thepresent model is identified with the spin-1/2ferromagnetat low enough temperatures.We note that the lowest-temperature QMC estimates,which successfully implythe T1/2asymptotic behavior of the specific heat and theT−2divergence of the magnetic susceptibility,were ob-tained through the improved algorithm[13]by spendingforty million MC steps on each data point.At mid temperatures in the specific heat,the antiferro-magnetic aspect most clearly appears.The specific heatexhibits a sharp peak,rather than a broad one charac-teristic of ferromagnets,at k B T/J≃0.74and thereforereminds us of the Schottky anomaly peculiar to the anti-ferromagnetic specific heat[13,14,16,17].It is interestingtofit the QMC result to the Schottky-type specific heatC2k B T2sech2 ∆3k B T kσ=± nσk(1+ nσk),(10)with n±k =[e(ω±k±µ)/k B T−1]−1,whereµis a Lagrangemultiplier determined by the condition(8).The suscep-tibility has been obtained by calculating the thermal av-erage of M2[18].Equations(9)and(10)are expanded in powers of t1/2at low temperatures and result inC4 S−s2ζ(3√2−132(S−s)12 (S2+Ss+s2)ζ(5√2)2π t3N(gµB)2=Ss(S−s)22(S−s)32)2πt-32)2π2t-1+O(t-1ωk=0,(13)where the normal ordering is taken with respect toαandβ.The spin-wave theory shows us that the clas-sical staggered magnetization(S+s)N is modified into (S+s)N−2τwith a quantum spin reductionτ[5].Equa-tion(13)claims that the thermalfluctuation of the stag-gered magnetization be constrained to take the classical value.This is analogous to Eq.(8),which claims that the thermalfluctuation of the magnetization be the classical magnetization(S−s)N.We stress that the constraint (13)leads in fact to exactly the same expressions as Eqs.(11)and(12)at low temperatures.Now we again obtaina set of self-consistent equations:F=E g+µ(S+s)N−k B T k σ=±ln(1+ nσk),(14)χ=(gµB)2through the Grant-in-Aid09740286and by a Grant-in-Aid from the Okayama Foundation for Science and Tech-nology.Most of the numerical computation was done us-ing the facility of the Supercomputer Center,Institute for Solid State Physics,University of Tokyo.0.01.02.03.04.05.06.0C / N k Bk B T / JFig.1(a)0.01.02.03.04.05.06.0χJ /N g 2µB2k B T / JFig.1(b)C / N k Bk B T / JFig.2(a)0.01.02.03.04.05.06.0χ k B T /N g 2µB2k B T / JFig.2(b)。
