Measurement of the open-charm contribution to the diffractive proton structure function
Synchronization

Synchronization“Synchronised”redirects here.For the racehorse,see Synchronised(horse).Synchronization is the coordination of events toop-Firefighters marching in a paradeerate a system in unison.The familiar conductor of an orchestra serves to keep the orchestra in time.Systems operating with all their parts in synchrony are said to be synchronous or in sync.Today,synchronization can occur on a global basis through the GPS-enabled timekeeping systems(and sim-ilar independent systems operated by the EU and Russia). 1TransportTime-keeping and synchronization of clocks was a crit-ical problem in long-distance ocean navigation;accurate time is required in conjunction with astronomical obser-vations to determine how far East or West a vessel has traveled.The invention of an accurate marine chronome-ter revolutionized marine navigation.By the end of the 19th century,time signals in the form of a signal gun,flag, or dropping time ball,were provided at important ports so that mariners could check their chronometers for error. Synchronization was important in the operation of19thcentury railways,these being thefirst major means of transport fast enough for the differences in local time be-tween adjacent towns to be noticeable.Each line han-dled the problem by synchronizing all its stations to head-quarters as a standard railroad time.In some territories, sharing of single railroad tracks was controlled by the timetable.The need for strict timekeeping led the compa-nies to settle on one standard,and civil authorities even-tually abandoned local mean solar time in favor of that standard.2CommunicationIn electrical engineering terms,for digital logic and data transfer,a synchronous circuit requires a clock signal. However,the use of the word“clock”in this sense is dif-ferent from the typical sense of a clock as a device that keeps track of time-of-day;the clock signal simply sig-nals the start and/or end of some time period,often very minute(measured in microseconds or nanoseconds),that has an arbitrary relationship to sidereal,solar,or lunar time,or to any other system of measurement of the pas-sage of minutes,hours,and days.In a different sense,electronic systems are sometimes synchronized to make events at points far apart appear si-multaneous or near-simultaneous from a certain perspec-tive.(Albert Einstein proved in1905in hisfirst relativ-ity paper that there actually are no such things as abso-lutely simultaneous events.)Timekeeping technologies such as the GPS satellites and Network Time Protocol (NTP)provide real-time access to a close approximation to the UTC timescale and are used for many terrestrial synchronization applications of this kind. Synchronization is an important concept in the following fields:•Computer science(In computer science,especiallyparallel computing,synchronization refers to the co-ordination of simultaneous threads or processes tocomplete a task with correct runtime order and nounexpected race conditions.)•Cryptography•Multimedia•Music(Rhythm)•Neuroscience124SEE ALSO•Photography•Physics(The idea of simultaneity has many difficul-ties,both in practice and theory.)•Synthesizers•Telecommunication3Uses•Film synchronization of image and sound in sound film.•Synchronization is important infields such as digital telephony,video and digital audio where streams of sampled data are manipulated.•In electric power systems,alternator synchronization is required when multiple generators are connected to an electrical grid.•Arbiters are needed in digital electronic systems such as microprocessors to deal with asynchronous inputs.There are also electronic digital circuits called synchronizers that attempt to perform arbi-tration in one clock cycle.Synchronizers,unlike arbiters,are prone to failure.(See metastability in electronics).•Encryption systems usually require some synchro-nization mechanism to ensure that the receiving ci-pher is decoding the right bits at the right time.•Automotive transmissions contain synchronizers that bring the toothed rotating parts(gears and splined shaft)to the same rotational velocity before engaging the teeth.•Film,video,and audio applications use time code to synchronize audio and video.•Flash photography,see Flash synchronization Some systems may be only approximately synchronized, or plesiochronous.Some applications require that relative offsets between events be determined.For others,only the order of the event is important.4See also•Asynchrony•Atomic clock•Clock synchronization•Data synchronization•Double-ended synchronization•Einstein synchronization•Entrainment•File synchronization•Flywheel•Homochronous•Kuramoto model•Mutual exclusion•Neural synchronization•Phase-locked loops•Phase synchronization•Reciprocal socialization•Synchronism•Synchronization(alternating current)•Synchronization in telecommunications •Synchronization of chaos •Synchronization rights•Synchronizer•Synchronous conferencing•Time•Timing Synchronization Function(TSF)•Time transfer•Timecode•Tuning forkOrder synchronization and related topics•Rendezvous problem•Interlocking•Race condition•Concurrency control•Room synchronization•Comparison of synchronous and asynchronous sig-nallingVideo and audio engineering•Genlock•Jam sync•Word sync3 Aircraft gun engineering•Synchronization gearCompare with•Synchronicity,an alternative organizing principle tocausality conceived by Carl Jung.5References6External links•J.Domański“Mathematical synchronization of im-age and sound in an animatedfilm”47TEXT AND IMAGE SOURCES,CONTRIBUTORS,AND LICENSES 7Text and image sources,contributors,and licenses7.1Text•Synchronization Source:https:///wiki/Synchronization?oldid=686676536Contributors:The Anome,Waveguy,Heron, B4hand,Patrick,Michael Hardy,Kku,Meekohi,Karada,Iluvcapra,CesarB,Egil,Mac,Mulad,Colin Marquardt,AHands,Hyacinth, Grendelkhan,Robbot,Altenmann,Ancheta Wis,DavidCary,Pne,Nickptar,Zondor,JTN,Noisy,ArnoldReinhold,Dbachmann,Mwanner, Shanes,Guettarda,Liao,Richard Harvey,DanGunn,Wtshymanski,Gpvos,Ruud Koot,Graham87,Rjwilmsi,Vegaswikian,Ian Dunster, Wavelength,Hillman,Cascadian,DanMS,Yamara,Nicke L,CarlHewitt,Aldenhoot,Howcheng,Daniel Mietchen,Scottfisher,Closed-mouth,SmackBot,Ianwri,Rentier,Telestylo,Michaelll,SynergyBlades,Oli Filth,Dual Freq,UNV,ZachPruckowski,Izhikevich,Clean-wiki,Lambiam,ElectronicsPerson,16@r,Halaqah,Citicat,Kvng,Dre.velation2012,Alexignatiou~enwiki,Corpx,SymlynX,Epbr123, Marek69,NigelR,Nick Number,Peterhawkes,JEBrown87544,AntiVandalBot,Squidfishes,JAnDbot,Stijn Vermeeren,Jim.henderson, Speck-Made,Javawizard,Maurice Carbonaro,JohnGrantNineTiles,Edvige,Soundofmusicals,3p1416,Movedgood,Radagast3,Biscuittin, Vektor330,Pcontrop,Vice regent,ClueBot,Tigerboy1966,Mild Bill Hiccup,Mumiemonstret,PixelBot,Brews ohare,Galzigler,Poco a poco,Mabdul,Тиверополник,Numbo3-bot,Jarble,Luckas-bot,Jalal0,AnomieBOT,Materialscientist,ArthurBot,TheAMmollusc,Nas-nema,Subviking,FrescoBot,RedBot,MastiBot,TjeerdB,Meatball91,EmausBot,Donner60,ChuispastonBot,ClueBot NG,Synchrony-creator,MerlIwBot,Wbm1058,TejasDiscipulus2,EricEnfermero,Makecat-bot,Cerabot~enwiki,TwoTwoHello,Reatlas,Janek316,Kas-parBot and Anonymous:1107.2Images•File:Firefighters_in_Parade.jpg Source:https:///wikipedia/commons/d/d8/Firefighters_in_Parade.jpg License: CC0Contributors:/cdm4/item_viewer.php?CISOROOT=/p15195coll32&CISOPTR=68&DMSCALE=12.5& DMWIDTH=600&DMHEIGHT=600&DMMODE=viewer&DMTEXT=&REC=10&DMTHUMB=1&DMROTATE=0Original artist: Unknown•File:MontreGousset001.jpg Source:https:///wikipedia/commons/4/45/MontreGousset001.jpg License:CC-BY-SA-3.0Contributors:Self-published work by ZA Original artist:Isabelle Grosjean ZA•File:Question_book-new.svg Source:https:///wikipedia/en/9/99/Question_book-new.svg License:Cc-by-sa-3.0 Contributors:Created from scratch in Adobe Illustrator.Based on Image:Question book.png created by User:Equazcion Original artist:Tkgd2007•File:Wiktionary-logo-en.svg Source:https:///wikipedia/commons/f/f8/Wiktionary-logo-en.svg License:Public domain Contributors:Vector version of Image:Wiktionary-logo-en.png.Original artist:Vectorized by Fvasconcellos(talk·contribs), based on original logo tossed together by Brion Vibber7.3Content license•Creative Commons Attribution-Share Alike3.0。
The role of the hippocampus in solving the Morris water maze

Communicated by Mikhail Tsodyks The Role of the Hippocampus in Solvingthe Morris Water MazeA.David RedishDavid S.TouretzkyComputer Science Department and Center for the Neural Basis of Cognition, Carnegie Mellon University,Pittsburgh,P A15213-3891,U.S.A.We suggest that the hippocampus plays two roles that allow rodents to solve the hidden-platform water maze:self-localization and route replay.When an animal explores an environment such as the water maze,the combination of placefields and correlational(Hebbian)long-term poten-tiation produces a weight matrix in the CA3recurrent collaterals such that cells with overlapping placefields are more strongly interconnected than cells with nonoverlappingfields.When combined with global inhi-bition,this forms an attractor with coherent representations of position as stable states.When biased by local view information,this allows the animal to determine its position relative to the goal when it returns to the environment.We call this self-localization.When an animal traces specific routes within an environment,the weights in the CA3recurrent collaterals become asymmetric.We show that this stores these routes in the recurrent collaterals.When primed with noise in the absence of sensory input,a coherent representation of position still forms in the CA3population,but then that representation drifts,retracing a route.We show that these two mechanisms can coexist and form a basis for memory consolidation,explaining the anterograde and limited retrograde amnesia seen following hippocampal lesions.1Amnesia Following Hippocampal LesionsHippocampal lesions in humans produce devastating impairments in declar-ative memory(memories of specific items,events,or episodes)(Scoville &Milner,1957;Squire&Zola-Morgan,1988;Cohen&Eichenbaum,1993; Zola-Morgan&Squire,1993).Although these patients perform immediate recall tasks normally,they are strongly impaired at times greater than a few minutes.In addition to the anterograde amnesia,these amnesias extend backward in time to recently before the occurrence of the lesion,but they leave early memories intact(Squire&Zola-Morgan,1988).Similar results have been seen in nonhuman primates with hippocampal lesions(Squire& Zola-Morgan,1988;Zola-Morgan&Squire,1993).Neural Computation10,73–111(1998)c 1997Massachusetts Institute of Technology74 A.David Redish and David S.TouretzkyThe theory proposed to explain these data is that the hippocampus serves as a temporary store for memory(Marr,1969;Buzs´a ki,1989;Cohen& Eichenbaum,1993;McClelland,McNaughton,&O’Reilly,1995).However, no models of hippocampal function in specific memory tasks exist;all pub-lished models of declarative memory demonstrate storage and retrieval of arbitrary binary vectors(Marr,1971;Rolls,1989;Gluck&Myers,1993;Al-varez&Squire,1994;Hasselmo&Schnell,1994;O’Reilly&McClelland, 1994;Levy,1996;Rolls,1996).Although these theories can address general principles involved in memory,they cannot address the role of the hip-pocampus in specific tasks.This makes it difficult to compare their results to real experiments or to generate testable predictions from them.Anterograde and limited retrograde amnesias after hippocampal lesion are also seen in rats tested in the Morris water maze(Sutherland&Hoesing, 1993).The Morris water maze consists of a submerged platform placed somewhere within a pool of water made opaque with milk or chalk(Mor-ris,Garrud,Rawlins,&O’Keefe,1982).When placed in this pool,rats try to find a way out;they initially swim randomly until theyfind the platform and climb out.Normal rats quickly learn the location of the platform:if the platform is removed,the rats search at the place where the platform had been(Morris et al.,1982).Rats with hippocampal lesions cannot learn this task(Morris et al.,1982;Morris,Schenk,Tweedie,&Jarrard,1990;McDon-ald&White,1994).If the rats are trained on the taskfirst and then given a hippocampal lesion1week later,they show profound deficits;however,the same lesion12weeks after training produces much smaller deficits(Suther-land&Hoesing,1993).Here,then,is a specific amnesia result that can be modeled in detail.2Modeling Memory in the Morris Water MazeWe suggest that rats trained on the Morris water maze use two different mechanisms tofind the hidden platform,one locale based and one route based.The two mechanisms can be subdivided intofive steps,thefirst two of which are locale based and the last three route based.Thesefive steps occur in order(we address possible ways to sidestep this in section4.2):1.Exploration.The animal familiarizes itself with the environment.2.Self-localization.Upon reentry into the environment,the animal mustdetermine its location relative to the platform.From this information, it can determine the direction it needs to swim in order to reach the platform.3.Route learning.When the animal travels along a specific path,routesare stored in the recurrent connections of hippocampal area CA3.4.Replay of routes during sleep.During sleep,the recent routes are replayedbecause,when primed with noise,the hippocampal formation settlesThe Role of the Hippocampus75 to a stable representation of a location,which then drifts along the routes stored in the CA3recurrent connections.McNaughton,Skaggs, and Wilson(Wilson&McNaughton,1994;Skaggs&McNaughton, 1996)have reported data supporting this hypothesis:simultaneous ex-tracellular recordings from hippocampal pyramidal cells have shown that cells tend tofire in the same sequence during slow-wave sleep as they did during recent experience in an environment.We discuss this and its implications for the theory in section2.4.5.Consolidation.The“dreamed”routes are transferred to long-term stor-age by a slowly learning cortical network.Anterograde amnesia occurs because long-term memory requires a hip-pocampus in order to learn the routes.Retrograde amnesia occurs when long-term memory has not been completely trained at the time the hip-pocampus is lesioned.Once the routes have been stored in long-term mem-ory,the animal can solve the task without a hippocampus.Our previous work laid out a theory of the role of the hippocampus in navigation(Touretzky&Redish,1996;Redish&Touretzky,1997)(see Figure1).The key components of the expanded theory presented here are as follows:•Path integration occurs via a loop including the superficial layers of the entorhinal cortex(ECs),the subiculum(Sub),and the parasubiculum (PaS).1•Sensory cues(called the local view,but not solely visual)enter the hippocampal formation from high-level sensory association areas,re-ferred to here as HLS.•The path integration and local view representations arefirst combined in ECs,but any conflicts are resolved by the recurrent connections in CA3.•On reentry into a familiar environment,competitive dynamics in the hippocampus allows the system to settle to a coherent place code even with ambiguous sensory cues.2This coherent code resets the path inte-grator so that multiple experiences of the same environment are com-patible with each other.•During sleep,recurrent connections within the hippocampus force a coherent code to form from noise,but due to asymmetric connection strengths produced during training,the represented location precesses 1Path integration is the ability to return to a starting point,even after a long,circuitous path,using only idiothetic cues(Mittelstaedt&Mittelstaedt,1980;Gallistel,1990;Etienne, 1992).2The place code is coherent if all neural activities are consistent with a representation of the same location in space.76 A.David Redish and David S.TouretzkyThe Role of the Hippocampus77 two modes.During motion,in the presence of ACh,the hippocampal EEG shows a7–12Hz rhythm called theta;during rest and slow-wave sleep,in the absence of ACh,the hippocampal EEG shows irregular activity,called LIA(large-amplitude irregular activity),characterized by short-duration sharp waves(Vanderwolf,1971).We now proceed to detail how our theory accounts for each of thefive steps discussed above,reviewing the data supporting the theory and sim-ulation results demonstrating each point.Additional simulation details are given in appendix A.2.1Exploration:Learning the Cognitive Graph.We begin by showing that the combination of random exploration and correlational(Hebbian) learning produces a weight matrix in the CA3recurrent connections that is appropriate for the competitive dynamics needed for self-localization.Fol-lowing Muller,Kubie,and Saypoff(1991),we call this connection function the cognitive graph:the synaptic efficacies between place cells are inversely related to the distance between the centers of their placefields.As the animal wanders around the environment,cells with nearby place fields are more likely to be coactive than cells with well-separatedfields. Combined with correlational long-term potentiation(LTP),in which the synaptic efficacy is increased when both cells are simultaneously active, after a session of wandering around an environment,the intrahippocampal connections will be inversely proportional to the distance between place field centers(Muller et al.,1991).LTP has been shown extensively in the recurrent connections in CA3and in the Schaffer collaterals connecting CA3 to CA1(see Landfield&Deadwyler,1988).Also supporting this theory are data showing the effect of ACh:while suppressing neuronal transmission in intrahippocampal synapses,ACh en-hances LTP in them(Hasselmo&Schnell,1994).We make the simplifying assumption that ACh shuts off the CA3recurrent connections completely. Experiments in hippocampal slices show that it diminishes synaptic efficacy across the Schaffer collaterals by approximately90percent,while diminish-ing the efficacy of the perforant path(inputs from ECs)by only approxi-mately45percent(Hasselmo&Schnell,1994).ACh presumably is present during theta mode,while the animal is moving about the environment.Dis-ruption of ACh has been found to shift the hippocampus out of theta,while cholinergic agonists shift the hippocampus into theta mode(Huerta&Lis-man,1993).LTP produced by hippocampal stimulation during theta or at intervals corresponding to the theta frequency is much stronger than similar stimulation during nontheta(LIA)(Larson,Wong,&Lynch,1986;Larson &Lynch,1989;Greenstein,Pavlides,&Winson,1988;Pavlides,Greenstein, Grudman,&Winson,1988;Huerta&Lisman,1993).Simulations.The network used to demonstrate the generation of the cognitive graph consisted of a limited version of the total model presented78 A.David Redish and David S.Touretzky in Figure1.It included HLS,PI,HD,ECs,and CA3/1components.Specific neuronal model details are given in appendix A.The HD component consisted of a1D(circular)neural array.At every time step,the currently represented value was calculated by a directional mean,the represented value was updated by the angular velocity,and then a new(idealized)HD representation was generated.This allowed us to simulate the neural interactions between the head direction and other rep-resentations without the computational time required to simulate the ac-tual head direction update mechanism.We have previously shown that a postsubiculum-anterior thalamic nuclei head direction model can track real angular velocity sequences accurately(Redish,Elga,&Touretzky,1996). The PI simulation was similar but used a two-dimensional(2D)(toroidal) neural sheet.We also simulated the HLS component as a bump on a2D neural sheet(as in Samsonovich&McNaughton,in press)and assumed that at every point in the environment,the position of the animal was correctly represented by the population.We did this because it is not clear what aspects of the environment are included in the local view;any set of spatial information about landmarks sufficient to localize the animal to a point will do.For some experiments,such as those described in section2.2,there was more than one peak in the local view representation.This allowed us to ask questions about the ability of the system to handle ambiguous inputs without having to build a complicated,speculative model of the visual system of the rodent.The EC’s population was also simulated as a2D neural sheet receiving input from the HLS and PI components.Because we do not separately simulate CA3and CA1,we refer to the combined simulated population as CA3/1but refer to CA3and CA1in our discussions of the theory proper.The CA3/1population consisted of a2D neural sheet connected to the PI representation by one-to-one connections.A sparse random connection pattern works just as well,but by using a one-to-one pattern,we know the center of the placefield for each CA3neuron.According to our theory,every time an animal enters an environment,it self-localizes as best it can by a sharp wave(LIA mode).We do not measure EEG in our simulations,but the self-localization sequence begins with a high proportion of the CA3cells active at low levels,and settles to a small number of highly active cells within approximately100ms(see section2.2). We believe this corresponds to a single sharp wave(Buzs´a ki,1989).We thus begin exploration byfirst placing the simulated animal at a random location and triggering a100-ms sharp wave.Since this is a novel environment,there are no stored associations in the intrahippocampal con-nections,and the sharp wave serves only to reset the path integrator to a random point.This random location becomes the reference point for the en-vironment,and the origin for the path integrator coordinate system.The an-imal then explores the environment by wandering randomly,during which LTP occurs in the intrahippocampal connections.The Role of the Hippocampus79Figure2:Route traveled by simulated rodent while exploring a100-cm diameter circular environment for5minutes.Dots indicate position sampled every10 seconds.Gray area denotes arena.Two effects must occur for the animal to have familiarized itself with the environment.First,a mapping from local views(in HLS)to path inte-grator coordinates(in ECs)must be learned.Second,because local views may be ambiguous,the cognitive graph must be learned in the recurrent connections of CA3.We show that the appropriate connection function appears within thefirst minutes of exploration.Figure2shows the track of the simulated animal wandering over the environment during the5minutes of exploration.The animal has clearly covered the entire environment.Figure3shows a scatter plot of the learned synaptic weights as a function of the distance between each pair of units in the CA3/1population.The synaptic efficacy between two cells is,on average,inversely related to the distance between the centers of their placefields.A similar plot of HLS-to-ECs synapse strengths would show that local view representations(in HLS)have been associated with path integrator coordinates(in ECs).Although the connection function appears quickly in our simulations,we used very large learning rates in order to minimize computation time.We do not know whether more realistic learning rates would allow the function to be acquired so quickly.If they did,the random trajectories shown by80 A.David Redish and David S.TouretzkyFigure3:Scatter plot of learned synaptic weights as a function of distance be-tween pairs of units.Distance is in cm.Line indicates the mean.animalsfirst placed in the water maze(with no knowledge of the goal location)would be sufficient to“explore”the environment.2.2Self-Localization and Place Field Stability.In order to navigate within a familiar environment,an animal must use a consistent representa-tion of position from session to session.Although visual cues can serve to inform the animal of its initial position,if they are ambiguous,there must be a mechanism to settle on a consistent representation of location.We be-lieve intrinsic competitive dynamics force the hippocampus to settle on a coherent code.These dynamics can serve as a disambiguation mechanism and can reproduce the search pattern that gerbils make when faced with ambiguous local cues(Touretzky&Redish,1996).We suggest that the competitive dynamics realized in the rodent pro-ceeds thusly:subiculum,parasubiculum,hipppocampus,and entorhinal cortex are initially noisy;sensory cues in HLS passed through ECs into the hippocampus proper bias the randomfiring rates with candidate locations. The recurrent connections in CA3allow one of these candidate locations to win out,forming a coherent place code in hippocampus.The connec-tions between CA1and subiculum reset the path integrator to the correct representation of the animal’s location in path integrator coordinates.This happens in the course of a single sharp wave during LIA.In our simulations, the place code in CA3/1is coherent within50to100ms.Figure4shows the first70ms of a simulated sharp wave.The Role of the Hippocampus81Figure4:Starting from random noise,a coherent place code forms in less than 50ms.Plot showsfiring rates of CA3/1place cells.Cells are laid out in a2D sheet with their locations in the sheet corresponding to the centers of their place fields in the environment.Intensity values have been interpolated for clarity. White indicates highfiring rate,black low.82 A.David Redish and David S.TouretzkyDuring a sharpwave,place cells do not show normal placefields;many cells are simultaneously active(many more than would normally be active during theta)(Buzs´a ki,1989).Because ACh is not present,synaptic efficacy between CA3cells is presumably at full strength,allowing the system to settle from an initially noisy state to a coherent representation of the ani-mal’s location.Once this representation is coherent,the path integrator(in subiculum,receiving strong connections from CA1)is driven by the now co-herent representation of location in CA1and is effectively reset.The animal can now navigate around the environment.Simulations.The network used to demonstrate self-localization used a similar architecture to that set out in section2.1.The2D neural sheets were enlarged to20×20,and the hippocampal simulation was more detailed.We simulated the CA3/1population as two pools,one excitatory and one in-hibitory(labeled CAE and CAI,respectively,in appendix A).The excitatory neurons were interconnected within and between pools by an idealization of the connection function learned in section2.1(a gaussian with a standard deviation of20cm).We had to use an idealization because our networks are small relative to those in the actual rodent brain.Inhibitory CA3/1neurons were broadly connected to both the excitatory and inhibitory pools.Essen-tially,this connection structure corresponds to local excitation and global inhibition.We measured the ability of this self-localization process to handle ambi-guities in the local view by locking three bumps into the HLS representation. This simulates three“candidate positions”in the local view.This ambigu-ous local view representation is resolved in the CA3/1representation intoa coherent representation of position similar to that shown in Figure4.2.3Route Learning.Given a representation of the animal’s current loca-tion in the environment and a representation of the current goal,the animal should be able to calculate the direction to take to reach the goal.The nu-cleus accumbens receives information about current needs and desires from the amygdala(Davis,Rainnie,&Cassell,1994)and information about cur-rent location via the fornix(Witter,Ostendorf,&Groenwegen,1990)and is optimally situated to perform this function.This function of the nucleus accumbens wasfirst suggested by Mogenson(1984),and a model showing its feasibility has been presented by Brown and Sharp(1995).(See Redish and Touretzky(1997)for a review.)There are three neurophysiological effects that allow the hippocampus to store routes as the animal travels.First,the postsynaptic potential(PSP) has a nonzero time constant.As an animal travels from the placefield of one neuron(say,a)to another(say,that of neuron b),neuron a continues to have an effect on thefiring rate of neuron b,but when the animal was in placefield a,neuron b did not have an effect on neuron a.Second,imagine the animal at an instant along the route taken,passingThe Role of the Hippocampus83 through a placefield centered slightly off the route.This cell will have a firing rate somewhere between its maximum and minimumfiring rates. Cells with placefields closer to the animal’s position will have higherfiring rates,and cells with placefields farther will have lower rates.This means that the output connection function from the neuron in question will be biased asymmetrically toward the path traveled.Finally,as the animal moves through the placefield,the timing of the spikesfired by that cell precesses with respect to the theta rhythm:cells behind the animalfire early in the cycle,while cells ahead of the animal fire late in the cycle(O’Keefe&Recce,1993;Skaggs,1995;Skaggs&Mc-Naughton,1996;Skaggs,McNaughton,Wilson,&Barnes,1996).Thus the represented position sweeps across the actual position from back to front with each theta cycle.When combined with the biophysical time course of LTP,this phase precession will also favor connections pointing along routes to a goal(Blum&Abbott,1996).Simulations.The route-learning simulation consisted of the same net-work as used in section2.2,with the addition of a new hippocampal mode. The simulation parameters as described in section2.2correspond to LIA mode,while the simulation parameters used for the route-learning simula-tion correspond to theta mode(see appendix A).We do not explicitly model the nucleus accumbens.Instead we compare the subicular representation at the goal and the current subicular represe-nation,and then simulate travel in a straight line until the animal reaches either the goal or a wall.Figure5shows the paths traveled to reach the goal from the four cardinal points.These are the four routes that will be stored in the CA3/1population.We model the asymmetric nature of LTP by making the learning rule dependent on the synaptic drive of the presynaptic neuron and thefiring rate of the postsynaptic neuron(see equation A.4).The synaptic drive S i of neuron i is the effect of that neuron on all the neurons on which it synapses divided by the synaptic weight over each synapse(Pinto,Brumberg,Simons, &Ermentrout,1996;see appendix A).It can be understood as a model of the postsynaptic potential or as a decaying memory of recentfiring rates shown by neuron i,with a decay time constant ofτi.We do not model phase precession as an emergent result of a complex process;instead we assume that phase precession exists and show that, when combined with the asymmetric temporal nature of LTP,routes are stored in the recurrent connections of the hippocampus.In order to produce phase precession,we derive the preferred phase of each CA3neuron using the approximation in Figure6.We then define thefiring rate of each neuron at time t asF i(t)=e−(˜θi(t)−θ(t))2/ρ2·ˆF i(t),(2.1)84 A.David Redish and David S.TouretzkyFigure5:Four routes to the goal location.In order to demonstrate the accuracy of the simulation,the direction to the goal was determined by comparing the representation in subiculum with the prior subicular representations of the goal location.Lines indicate trajectories taken by the simulated animal to reach the goal(indicated by small circle).An x has been drawn at the initial location of the animal in each position.These routes are stored in the CA3/1structure via LTP.Gray area denotes arena.where˜θi(t)is the preferred phase of neuron i,θ(t)is the current phase of the theta rhythm,ρis a constant,andˆF i(t)is the peakfiring rate at θ(t)=˜θ(t).We assume a theta rhythm with a frequency of7Hz,soθ(t)=(7·360◦sec ·t)mod360◦.ˆF i(t)is determined by equation A.2(see appendix A).This makes the representation of position sweep from behind the animal to in front of it with each theta cycle as it does in the real animal(O’Keefe &Recce,1993;Skaggs et al.,1996).We do not claim this as a model of how phase precession is actually generated in the rodent hippocampus,only that it produces a phase precession effect so that routes can be stored in the CA3 recurrent connections.These effects combine to store routes in the recurrent connections of CA3. They produce a vectorfield pointing toward the path and then leading to the goal.Figure7shows the routes stored by an animal traversing the four paths in Figure5.The Role of the Hippocampus85Figure6:How we simulated phase precession.Let L(t)be a ray originating at the simulated rodent’s current position(as represented by the pyramidal cells in CA3),pointing in the direction of its current heading(as represented by the cells in postsubiculum).Let P i(t)be a vector from the represented position of the rodent to the center of the placefield of place cell i,and D i(t)be the projectionof P i(t)onto L(t).Then the preferred phase of neuron i,˜θi(t)is proportional to D i(t):˜θi(t)=K·D i(t),where K is a scale factor chosen to be small enough that the phase precession will not wrap around(K=1.2deg/cm in our simulations). Thus,cells with placefields behind the represented position(in CA3)fire earlier in the theta cycle,and cells ahead of the represented positionfire later.We do not claim this as a model of how phase precession is actually generated in the rodent brain,only that it produces a phase precession effect so that routes can be stored in the CA3recurrent connections.2.4Replay of Routes During Sleep.When there is sensory input into the hippocampus and the hippocampus is in LIA mode(i.e.,the animal is awake and looking around but not moving),sensory cues enter the system via HLS and ECs,and those CA3cells that are consistent with the current local view will be more active than those that are not.This biases CA3to settle to a place code consistent with the local view and thus can serve as a self-localization procedure.On the other hand,when there is no sensory input,this bias will be absent,but due to the recurrent connections in CA3,the hippocampus will still settle into a coherent activity pattern.Due to the asymmetric connections that were stored when the animal traversed the routes to the goal,the place86 A.David Redish and David S.TouretzkyFigure7:Vectorfield of routes to a goal stored in the recurrent connections of the model CA3.For each cell j,we calculated the center of mass of the output connection weights,and plotted an arrow from the placefield center toward the center of mass.Length of arrow corresponds to linearly scaled distance to center of mass of the output connection weights.code will precess along one of these remembered routes.The bias provided by the sensory input should be enough to keep the system from precessing when awake,but in the absence of sensory input(during sleep),there is nothing to stop the precession.During sleep,when sharp waves occur without sensory input,we expect to see replay of routes.This is shown in Figure8.Given an initial noisy state,a coherent code forms within half a second,and then over the next few seconds,it drifts along a remembered route.Data supporting a replay of recent experience in hippocampus during sleep werefirst seen by Pavlides and Winson(1989).They showed that cells with recently visited placefields were more active during REM sleep than other cells whose placefields had not been recently visited.Wilson andThe Role of the Hippocampus87Figure8:Replay of routes during LIA without sensory input.A coherent code forms quickly and then slowly drifts to the goal over the subsequent few seconds. McNaughton(1994)showed that during slow-wave sleep(SWS),cells that showed correlatedfiring during a session in an environment(because their placefields overlapped)also showed a stronger correlation during sleep immediately after the session.Skaggs and McNaughton(1996)explicitly examined the temporal nature of replay during sharp waves in slow-wave sleep.They defined the temporal bias B ij between two cells i and j to be the difference between the integrated cross-correlation for the200ms after each spike of cell j and the integrated cross-correlation for the200ms before each spike of cell j.Thus,if cell i generallyfires after cell j rather than before,B ij will be greater than0.。
LABORATORY MANUAL 9.1 SPRING CONSTANT说明书
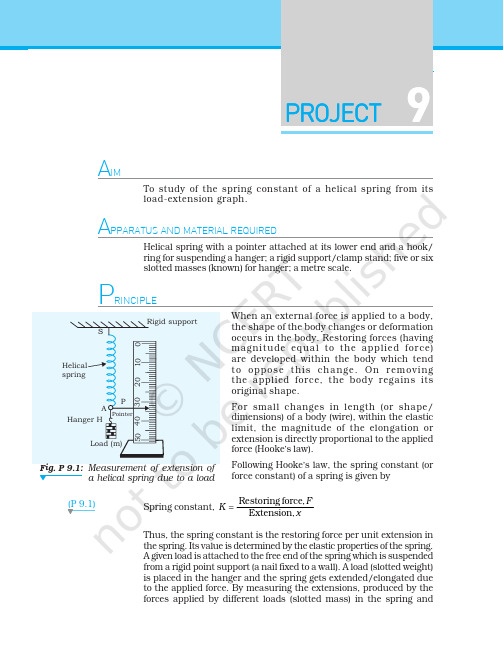
208PROJECT9 PointerAHanger HHelicalspringLoad (m)Rigid support12345SPFig. P 9.1:Measurement of extension ofa helical spring due to a load(P 9.1)A IMTo study of the spring constant of a helical spring from itsload-extension graph.A PPARATUS AND MATERIAL REQUIREDHelical spring with a pointer attached at its lower end and a hook/ring for suspending a hanger; a rigid support/clamp stand; five or sixslotted masses (known) for hanger; a metre scale.P RINCIPLEWhen an external force is applied to a body,the shape of the body changes or deformationoccurs in the body. Restoring forces (havingmagnitude equal to the applied force)are developed within the body which tendto oppose this change. On removingthe applied force, the body regains itsoriginal shape.For small changes in length (or shape/dimensions) of a body (wire), within the elasticlimit, the magnitude of the elongation orextension is directly proportional to the appliedforce (Hooke’s law).Following Hooke’s law, the spring constant (orforce constant) of a spring is given bySpring constant, =Restoring force,Extension,FKxThus, the spring constant is the restoring force per unit extension inthe spring. Its value is determined by the elastic properties of the spring.A given load is attached to the free end of the spring which is suspendedfrom a rigid point support (a nail fixed to a wall). A load (slotted weight)is placed in the hanger and the spring gets extended/elongated dueto the applied force. By measuring the extensions, produced by theforces applied by different loads (slotted mass) in the spring and209PROCEDURE1.Suspend the helical spring, SA, having a pointer, P, at itslower free end, A, freely from a rigid point support, as shown in Fig. P 9.1.2.Set the metre scale close to the spring vertically. Take care thatthe pointer moves freely over the scale without touching it and the tip of the pointer is in front of the graduations on the scale.3.Find out the least count of the metre scale. It is usually 1 mm or0.1 cm.4.Record the initial position of the pointer on the metre scale,without any slotted mass suspended from the hook.5.Suspend the hanger, H (of known mass, say 20 g) from the lowerfree end, A, of the helical spring and record the position of the pointer, P on the metre scale.6.Put a slotted mass on the hanger gently. Wait for some time forthe load to stop oscillating so as to attain equilibrium (rest)position, or even hold it to stop. Record the position of the pointer on the metre scale. Record observations in a table with proper units and significant figures.7.Put another slotted mass on the hanger and repeat Step 6.8.Keep on putting slotted masses on the hanger and repeat Step 6.Record the position of the pointer on the metre scale every pute the load/force F ( = mg ) applied by the slotted mass,M and the corresponding extension (or stretching), x in the helical spring. Here g is the acceleration due to gravity at the place of the experiment.10.Plot a graph between the applied force F along x-axis and thecorresponding extension x (or stretching) on the y-axis. What is the shape of the curve of the graph you have drawn?11.If you find that the force-extension graph is a straight line, findthe slope (F /x ) of the straight line. Find out the spring constant K of helical spring from the slope of the straight line graph.OBSERVATIONSLeast count of the metre scale= ... mm= ... cm Mass of the hanger = ... g210Mean Spring constant K = ... N/mPlotting load - extension graph for a helical springTake force, F along the x-axis and extension, x along the y-axis.Choose suitable scales to represent F and x . Plot a graph between F and x (as shown in Fig. P 9.2). Identify the shape of the load-extension graph OA.CALCULATIONSChoose two points, O and A, wide apart on the straight line OA obtained from load extension graph, as shown in Fig. P 9.2. Fromthe point A, draw a perpendicular AB on x-axis. Then, from the graph,Slope of the straight line graph = tan θ =ABOB= x /F Spring constant, K = Fx=1(slope of the graph)Spring constant, −===−–1B O A BOB ...Nm AB F F K x x where x A and x B are the corresponding extensions at points A and B (or O) respectively where F B and F O are the loads (forces) at points B and O.yOxAE x t e n s i o n (m )F (N)BS.No.Mass suspended from the spring, MForce,F = mgPosition of the pointerExtension,x Springconstant, K(= F /x )(10–3 kg)(N)(cm)(10–2 m)(N m –1)102203.4.5.6.........Fig. P 9.2: Load-extension graphfor a helical spring211R ESULTThe spring constant of the given helical spring = ... Nm –1PRECAUTIONS1.The spring should be suspended from a rigid support and itshould hang freely so that it remains vertical.2.Slotted weights should be chosen according to elastic limit ofthe spring.3.After adding or removing the slotted weight on the hanger, waitfor sometime before noting the position of the pointer on the scale because the spring takes time to attain equilibrium position.SOURCES OF ERROR1.If support is not perfectly rigid, some error may creep in due tothe yielding of the support.2.The slotted weights may not be standard weights.DISCUSSION1. A rigid support is required for suspending the helical spring withload (or slotted mass) from it. The slotted masses may not have exact values engraved on them. Some error in the extension is likely to creep in due to the yielding (sometimes) of the support and inaccuracy in the values of the masses of loads.2.The accuracy of the result depends mainly on the measurementof extension produced by the force (load) within the elastic limit.Take special care that the slotted mass is put gently on the hanger as the wire of the helical spring takes sometime to attain its new-equilibrium position.3.If the elastic limit is crossed slightly, what changes will you expectin the spring and your result?SELF ASSESSMENT1.Two springs A (of thicker wire) and B (of thinner wire) of the samematerial, loaded with the same mass on their hangers, are suspended from a rigid support. Which spring would have more value of spring constant?2.Soft massive spring of mass M s and spring constant K hasextension under its own weight. What mass correction factor for212attached at its lower end would be =+sm()()2MF gX MK K]3.What other factors affect spring constant, e.g. length.SUGGESTED ADDITIONAL EXPERIMENTS/ACTIVITIES1.Take spring of the same material but of different diameters of thewires. See how the spring contant varies.2.Take springs of the same diameters of the wires but of differentmaterials. See how the spring constant varies. What inference doyou draw from your result?。
gring wheel

Precision Engineering 27(2003)9–13Automatic compensation for grinding wheel wear by pressurebased in-process measurement in wet grindingKatsushi Furutani a ,∗,Nguyen Trong Hieu a ,1,Noriyuki Ohguro a ,2,Takashi Nakamura baDepartment of Advanced Science and Technology,Toyota Technological Institute,12-1,Hisakata 2-chome,Tempaku-ku,Nagoya 468-8511,Japanb Nagoya Institute of Technology,Gokiso-cho,Showa-ku,Nagoya 466-8555,JapanReceived 19December 2001;received in revised form 4June 2002;accepted 2July 2002AbstractThis paper deals with an application to automatic compensation of grinding wheel wear by a pressure based in-process measurement method in wet grinding.A pressure sensor is set beside a grinding wheel with a small gap.When grinding fluid is dragged into the gap by the rotation,hydrodynamic pressure,which corresponds to the gap length and the topography,can be measured.No electromagnetic properties of the workpiece and grinding wheel affect measured results.This method is applied to a CNC surface grinding machine.The pressure distribution and the relationship between the pressure and the gap length are measured.The pressure is decreased with the increase of the gap length.Its dispersion is around 1%for 0.5m-wear of a grinding wheel with 250m grit size.The dimensional error of a workpiece using the feedback of the wear is less than the feeding step,for the compensation,of 1m.©2002Elsevier Science Inc.All rights reserved.Keywords:Hydrodynamic pressure;Wear;In-process measurement;Repeatability;Compensation;Error1.IntroductionWet grinding is one of the major ways for high-precision machining.An error factor in grinding is wear of a grind-ing wheel.In order to reduce a machining error,in-process measurement of grinding wheel wear is required.A number of methods for the in-process measurement of the wear of a grinding wheel have been studied.An acoustic emission sensor is often used in contact measurement to detect the touch of a grinding wheel on a workpiece [1]or a sensing wire [2].This can be used for dry and wet grinding.How-ever,noncontact measurement is mainly used in the grinding process to avoid wear of a sensing target.A triangular sen-sor [3]and an air micrometer [4]have been used for dry grinding.Grinding fluid usually causes difficulty in the wear measurement of the grinding wheel.Therefore,the grinding fluid should be removed in most of the above methods.An ultrasonic sensor has been used for wet grinding by using the grinding fluid as a medium of ultrasonic waves [5].Its measurement accuracy is insufficient for precision grinding.∗Correspondingauthor.Tel.:+81-52-809-1796;fax:+81-52-809-1721.E-mail address:furutani@toyota-ti.ac.jp (K.Furutani).1Present address:Nagoya Institute of Technology,Gokiso-cho,Showa-ku,Nagoya 466-8555,Japan.2Present address:Sakai Plant,Daikin Industries,Ltd.,3–12,Chikuko-Shinmachi,Sakai,Osaka 592-8331,Japan.Some measurement methods by measuring static pressure caused by issuing fluid to a grinding wheel have also been proposed [6,7].Because these methods are similar to an air micrometer,superfluous grinding fluid must be removed for the accurate measurement.On the other hand,a grinding wheel drags grinding fluid into a small gap between a grinding wheel and workpiece during wet grinding so that hydrodynamic pressure is gen-erated in the gap [8].The authors have proposed a measure-ment method,which uses the hydrodynamic pressure of the grinding fluid to actively generate the pressure [9,10].In this paper,an in-process measurement method for the wear amount of a grinding wheel in a wet condition is intro-duced.The influence of grinding wheels and grinding condi-tions on the pressure is also investigated.Finally,the wear of a grinding wheel is compensated during grinding process.2.Principle of measurementFig.1shows the measurement principle applied to a sur-face grinding process [9,10].A pressure sensor is set beside a grinding wheel.Grinding fluid is fed towards the gap be-tween the grinding wheel and a pressure sensor.The grind-ing fluid is dragged by the rotation of the grinding wheel into the gap,generating hydrodynamic pressure in the gap.Any0141-6359/02/$–see front matter ©2002Elsevier Science Inc.All rights reserved.PII:S 0141-6359(02)00153-810K.Furutani et al./Precision Engineering27(2003)9–13Fig.1.Principle of measurement by using pressure. pressure change caused by the hydrodynamic pressure is mea-sured with the sensor.The hydrodynamic pressure decreases with the increase of the gap length between the sensor and the working surface of the grinding wheel.Once the relation-ship between the gap length and the pressure is calibrated, the gap length can be predicted by measuring the pressure. No electromagnetic properties of the grinding wheel,or the workpiece and grindingfluid affect the pressure.Viscosity of the grindingfluid seldom changes during grinding even if the temperature of the workingfluid is slightly changed or debris is present.The diameter change by the wear seldom affects the peripheral speed of the grinding wheel because the ratio of the wear to the wheel diameter is negligible.Therefore, the measurement process by this method becomes easier and more accurate than the measurement process with other non-contact sensors.Because the grindingfluid is actively used, no other additional device is needed.Grinding wheels with a diameter from205to355mm have been used in the experiments.Adequate output could be ob-tained over a peripheral speed of16m/s.The diameter of the pressure sensor must be smaller than the width of the grind-ing wheel[9–11].The roughness of the working surface of a grinding wheel changes because of loading,dulling and shedding during grinding,which affectsflow in the gap.As a surface becomes rougher,flow is separated earlier in general and becomes more turbulent.The topography change of the working sur-face of a grinding wheel can be detected by a frequency anal-ysis of the pressure[11].3.Experimental setupA CNC surface grinding machine is used in the follow-ing experiments.Fig.2shows an appearance of the ex-perimental setup around a grinding wheel and sensorunit.Fig.2.Appearance of experimental setup around grinding wheel and sensor unit.Table1Specifications of grinding conditionsMaximum revolution of grinding wheel40s−1Maximum peripheral speed25.8m/sResolution of depth of grinding0.1mTables1and2show the specifications of the grinding ma-chine and grinding wheels,respectively.Three grinding wheels composed of grains made from white fused alumina were used to grind normal carbon steel.The grinding wheels were balanced less than0.02m radial error dynamically. Each wheel had the same diameter of205mm in order to equalize the peripheral speed.Fig.3shows the arrangement of the pressure sensor,and the Cartesian coordinate system,which define the x-,y-and z-axes.The sensor is covered with a steel plate,with a hole of 6mm,to prevent direct contact with the grinding wheel.The surface roughness of the plate is1.7m R z.Table3shows specifications of the measurement instruments used in the experiments.The sensor with a diaphragm of6mm in di-ameter is a strain gauge type.The sensitivity of the pressure sensor is10kPa/V at the output of the strain amplifier.The pressure change is more important than the absolute pressure to measure wear,and a differential amplifier is sometimes used to amplify and shift the output of the pressure sensor. The sensor unit is mounted on the spindle column of the Table2Specifications of grinding wheelAbbreviation WA60WA120WA360 JIS code WA60G8V WA120J8V WA360J8V Average grain25012540size(m)Grade Very soft Soft SoftGrain White fused aluminaBond VitrifideWheel size(mm)205in outer diameterand19in widthGrain volume46ratio(%)K.Furutani et al./Precision Engineering27(2003)9–1311Fig.3.Arrangement of pressure sensor.Table3Specifications of measurement instrumentsType Strain gauge(diaphragm) Pressure sensor Nominal pressure100kPaDiameter of diaphragm6mmNatural frequency22kHzTemperature drift0.04%/KLinearity1%Strain amplifier Band width DC,200kHzAccuracy±0.1%of FSLinearity±0.01%of FS Differential amplifier Band width DC,10MHzMaximum offset voltage5VGain10–10000 FS:Full scale.grinding machine with a combination of an xyz stage driven by stepping motors.The gap is measured with an eddy cur-rent displacement sensor with a measurement range of1mm and a resolution of0.4m.Soluble-type grindingfluid containing surfactant is diluted 70times with water,and is supplied at aflow rate of6.7×10−5m3/s through a regulator for the measurement.4.Characteristics of generated pressureThe pressure is measured on the grinding machine to cal-ibrate the pressure to the gap length.Fig.4shows an ex-ample of the pressure at a rotational speed of2000min−1 (23.6m/s)and a minimum gap of0m for a W A60,shown in Table2.The output of the pressure sensor is amplified100 times and shifted with an offset of−2V with the differen-tial amplifier.A waviness of0.016m is observed in Fig.4a, and this corresponds to an unbalance of0.013m measured with a balance tester under dry condition.A significant fre-quency component of34Hz corresponding to therevolution Fig.4.Examples of pressure sensor output at2000rpm.(a)Time domain.(b)Frequency domain.of the grinding wheel is observed in Fig.4b.The pressure was at a maximum at x=−0.5mm at every gap length among 0,12and23m.Hereafter,the pressure is measured at this point.Fig.5shows relationships between the average of the pres-sure and the minimum gap length and the influence of the mesh size of grains.The minimum gap length is changed from 0to110m.With an increase of grain size or of the pores in the bond,the equivalent average gap length increases.Conse-quently,the pressure for a grinding wheel composed of large grains and a porous bond reduces at a faster rate even if the gap length is thesame.Fig.5.Influence of mesh size.Table2shows the specifications of WA60, WA120and WA360.12K.Furutani et al./Precision Engineering 27(2003)9–13Fig.6.Repeatability of measured pressure using WA60grinding wheel.Repeatability of the pressure was investigated when using a WA60grinding wheel.Fig.6shows the relation-ship between the average pressure and the minimum gap length,together with the difference between the maximum and minimum pressure measured at each gap.Results of five repetitive measurement are displayed in the figure.The sensitivity in this case is 0.178m/Pa.The maximum differ-ence between measured pressure and an average pressures is equivalent to a radial gap error of 0.5m up to a gap length of 70m.Because this error is the same as the resolution of the displacement sensor and the motion error of the stage,the error factors cannot be discriminated.pensation of grinding wheel wear 5.1.System configuration and algorithmFig.7shows the system configuration for automatic compensation of the grinding wheel wear byin-processFig.7.System configuration for automatic compensation by pressure based in-processmeasurement.Fig.8.Algorithm of automatic compensation of grinding wheel wear.FSC,feeding step for compensation;DG,depth of grinding.measurement.The pressure is measured with the pressure sensor and its output is magnified and shifted via the strain amplifier and the differential amplifier.The wear amount is then calculated using a personal computer,which gives the up/down command to the grinding machine.The NC con-troller returns the completion signal when the commanded feed is completed.The computer does not transfer the signal to the grinding machine until the completion signal is “off.”Fig.8shows an algorithm of the automatic compensa-tion process.Before machining commences,the relationship between the gap length and pressure,as shown in Fig.5,is calibrated by changing the position of the sensor.The wear increment is calculated according to the calibration curve during the grinding process.Once the wear increment exceeds the FSC,the spindle feed is adjusted accordingly.5.2.Result of compensationTable 4shows the experimental grinding conditions.The depth of grinding a pass and FSC were set to 3and 1m,respectively.JIS-SKD11(ASTM-D2)steel with a hardness of 42HRC was used for a workpiece to promote the wear of the grinding wheel.Fig.9shows the shape of the workpiece.Only the 15-mm wide surface is ground and the other 5-mm surface was used as a reference.The grinding depth was measured by comparing the heights of the surfaces with an electric comparator.K.Furutani et al./Precision Engineering 27(2003)9–1313Table 4Grinding conditionsGrinding method Traverse Peripheral speed23.6m/s Depth of grinding pass0.003mm Feeding step for compensation 0.001mm Traverse speed0.33m/s Number of spark out 10Grinding wheel JIS-WA60G8VWorkpiece JIS-SKD11(ASTM-D2)Hardness42HRCFig.9.Shape of testworkpiece.Fig.10.Progress of dimensional error of workpiece against grinding depth.Fig.10shows progress of dimensional error of a work-piece against grinding depth.The grinding amount is mea-sured every 30m as commanded by FSC.In the case of grinding without compensation,the dimensional error of the workpiece increases in proportion to the grinding wheel wear.However,in the case of grinding with compensation,the error is decreased to 0.5m.It takes the same time to grind with the automatic compensation as that without the compensation.6.ConclusionsWith the aim to improve the accuracy in the wet grinding,the grinding wheel wear was automatically compensated by the in-process measurement using the pressure generated by grinding fluid.Conclusions drawn were as follows:1.With the increase of the gap length,the pressure is de-creased.The porosity in the bond also affects the pressure.2.Repeatability of the measured wear by measuring the pres-sure is less than 1%within a small gap length of up to 70m.3.The dimensional error of the workpiece is less than the feeding resolution for a compensation of 1m.The authors can discriminate between wear of wheel diam-eter,dulling,shedding or loading,using the measured pres-sure during the actual grinding process.AcknowledgmentsThe authors wish to thank Professor Kazuyoshi Kondoh of Toyota Technological Institute (TTI)for his helpful advice,and Masaru Ohta and Susumu Kozakai of TTI and the staff of the TTI workshop for their helpful cooperation.This study is financially supported by Osawa Scientific Grant (OSG Fund),Fluid Power Technology Foundation and Grant-in-Aid for High-tech Research Center for “Space Robotics”and Aca-demic Frontier Center for “Future Data Storage Materials”by the Ministry of Education,Culture,Sports,Science and Technology,Japan.References[1]Dong WP,Annecchino L,Webster JA.On-line measurement of grind-ing wheel wear using acoustic emission.In:Proceedings of the 11th Annual Meeting of American Society for Precision Engineering,Monterey,CA,USA,1996.p.566–71.[2]Izumi M,Lee HS,Wakabayashi T,Inoue S.Development of a measur-ing instrument for grinding wheel peripheral shapes.In:Proceedings of the 15th Annual Meeting of American Society for Precision Engi-neering,Scottsdale,AZ,USA,Oct 2000.p.377–80.[3]Brinksmeier E,Werner F.Monitoring of grinding wheel wear.AnnCIRP 1992;41(1):373–6.[4]Schreitmüller HJ,Dederichs M.Pneumatisches Meßverfahrenzur Ermittlung des Schleif-scheibenverschleißes.Industrie-Anzeiger 1971;93(68):1733–4.[5]Spur G,Leonards F.Sensoren zur Erfassung von Prozesskenngrössenbei der Drehbearbeitung.Ann CIRP 1975;24(1):349–55.[6]Maksoud TMA,Mokbel AA,Morgan JE.In-process detection of grind-ing wheel truing and dressing conditions using a flapper nozzle ar-rangement,Proceedings of Institution of Mechanical Engineers.J Eng Manufact 1997;211(Pt B(5)):335–43.[7]Rahman JF,Radhakrishnan V .Measurement of grinding wheel surfacetopography using electro-pneumatic turbulence amplifier system.Int J Mach Tool Des Res 1980;20(3/4):189–96.[8]Arnell RD,Davies PB,Halling J.Tribology—principles and designapplications.London,UK:Macmillan Education,1991.[9]Furutani K,Katoh T,Mohri N.In-process measurement of wearof grinding wheel by using hydrodynamic pressure in wet grinding condition (1st report)—measurement principle.J Jpn Soc Prec Eng 2000;66(1):127–31.[10]Furutani K,Katoh T,Mohri N.In-process measurement of wear ofgrinding wheel by hydrodynamic pressure.In:Proceedings of the 14th Annual Meeting of American Society for Precision Engineering,Mon-terey,CA,USA,1999.p.610–3.[11]Furutani K,Ohguro N,Hieu NT,Nakamura T.Process measurementof topography of grinding wheel by using hydrodynamic pressure.Pro-ceedings of the 17th Annual Meeting of American Society for Precision Engineering,St Louis,MO,USA 2002;42(13):1447–53.。
中国民族乐器(英文版)

Guzheng (古筝)
A long, rectangular instrument with 21 strings. It is played by strumming the strings with a plectrum or plucking them with the fingers.
Erhu (二胡)
Chinese Ethnic Musical Instruments (English Versio
contents
目录
introduction The Types of Chinese Ethnic Musical Instruments The Role of Chinese Ethnic Musical Instruments in Music The influence and dissemination of Chinese ethnic musical instruments worldwide
Expression
Players must also learn to express emotions through their playing, as many Chinese ethnic musical pieces are designed to convey specific feelings or stories.
Stringed instruments
Douzi (鼔子)
01
A small, handheld percussion instrument made of clay or wood. It is played by holding it in one hand and striking it with the other hand.
Feasibility of Beauty Baryon Polarization Measurement in Lambda0 Jpsi Decay Channel by Atla

a rXiv:h ep-ph/945231v15May1994Institute of Physics,Acad.of Sci.of the Czech Rep.PRA–HEP–94/3and hep-ph/9405231Nuclear Centre,Charles University May 5,1994Prague Feasibility of Beauty Baryon Polarization Measurement in Λ0J/ψdecay channel by ATLAS-LHC Julius Hˇr ivn´a ˇc ,Richard Lednick´y and M´a ria Smiˇz ansk´a Institute of Physics AS CR Prague,Czech Republic submitted to Zeitschrift f¨u r Physik CAbstractThe possibility of beauty baryon polarization measurement by cascade decay angu-lar distribution analysis in the channel Λ0J/ψ→pπ−l +l −is demonstrated.The error analysis shows that in the proposed LHC experiment ATLAS at the luminosity 104pb −1the polarization can be measured with the statistical precision better than δ=0.010for Λ0b and δ=0.17for Ξ0b .IntroductionThe study of polarization effects in multiparticle production provides an important infor-mation on spin-dependence of the quark confinement.Thus substantial polarization of the hyperons produced in nucleon fragmentation processes[1,2]as well as the data onthe hadron polarization asymmetry were qualitatively described by recombination quark models taking into account the leading effect due to the valence hadron constituents[3−6].Although these models correctly predict practically zero polarization ofΛandΩ−,they fail to explain the large polarization of antihyperonsΣ−recently discovered in Fermilab[7,8].The problem of quark polarization effects could be clarified in polarization measure-ments involving heavy quarks.In particular,an information about the quark mass de-pendence of these effects could be obtained[4,9].The polarization is expected to be proportional to the quark mass if it arises due to scattering on a colour charge[10−12]. The opposite dependence takes place if the quark becomes polarized due to the interac-tion with an”external”confiningfield,e.g.,due to the effect of spontaneous radiation polarization[13].The decrease of the polarization with increasing quark mass is expected also in the model of ref.[14].In QCD the polarization might be expected to vanish with the quark mass due tovector character of the quark-gluon coupling[10].It was shown however in Ref.[15] that the quark mass should be effectively replaced by the hadron mass M so that even the polarization of ordinary hadrons can be large.The polarization is predicted to be independent of energy and to vanish in the limit of both low and high hadron transverse momentum p t.The maximal polarization P max(x F)is reached at p t≈M and depends on the Feynman variable x F.Its magnitude(and in particular its mass dependence)is determined by two quark-gluon correlators which are not predicted by perturbative QCD.The polarization of charm baryons in hadronic reactions is still unmeasured due to the lack of sufficient statistics.Only some indications on a nonzero polarization were reported[16,17].For beauty physics the future experiments on LHC or HERA give an opportunity to obtain large statistical samples of beauty baryon(Λ0b,Ξ0b)decays intoΛ0J/ψ→pπ−l+l−,which is favorable mode to detect experimentally.Dedicated triggers for CP-violation effects in b-decays,like the high-p t one-muon trigger(LHC)[18] or the J/ψtrigger(HERA)[19]are selective also for this channel.Below we consider the possibility of polarization measurement of beauty baryonsΛ0b andΞ0b with the help of cascade decay angular distributions in the channelΛ0b(Ξ0b)→Λ0J/ψ→pπ−l+l−.1Polarization measurement method and an estimation of the statistical error.In the case of parity nonconserving beauty baryon(B b)decay the polarization causes the asymmetry of the distribution of the cosine of the angelθbetween the beauty baryon decay and production analyzers:w(cosθ)=1| p inc× p B b|,where p inc and p Bbare momenta of incident particle and B b in c.m.system.The asymmetry parameterαb characterizes parity nonconservation in a weak de-cay of B b and depends on the choice of the decay analyzer.In the two-body decay B b→Λ0J/ψit is natural to choose this analyzer oriented in the direction ofΛ0momentum pΛ0in the B b rest system.The considered decay can be described by4helicity ampli-tudes A(λ1,λ2)normalized to unity:a+=A(1/2,0),a−=A(−1/2,0),b+=A(−1/2,1) and b−=A(1/2,−1),|a+|2+|a−|2+|b+|2+|b−|2=1.(2) The difference ofΛ0and J/ψhelicitiesλ1-λ2is just a projection of B b spin onto the decay analyzer.The decay asymmetry parameterαb is expressed through these amplitudes in the formαb=|a+|2−|a−|2+|b+|2−|b−|2.(3) If P-parity in B b decay were conserved,then|a+|2=|a−|2,|b+|2=|b−|2so thatαb would be0.In the case of known and sufficiently nonzero value ofαb the beauty baryon polarization could be simply measured with the help of angular distribution(1)(see, e.g.,[20]).Due to lack of experimental information and rather uncertain theoretical estimates ofαb for the decayΛ0b→Λ0J/ψ[21]both the polarization andαb(or the decay amplitudes)should be determined simultaneously.This can be achieved with the2help of information onΛ0and J/ψdecays.Though it complicates the analysis,it shouldbe stressed that the measurement of the beauty baryon decay amplitudes could give valuable constrains on various theoretical models.Generally,such a measurement canbe done provided that at least one of the secondary decays is asymmetric and its decayasymmetry parameter is known[9].In our case it is the decayΛ0→pπ−with the asymmetry parameterαΛ=0.642.The angular distribution in the cascade decay B b→Λ0J/ψ→pπ−l+l−follows di-rectly from Eq.(6)of[9],taking into account that the only nonzero multipole parameters.It can be written in the formin the decay J/ψ→l+l−are T00=1and T20=110w(Ω,Ω1,Ω2)=1formula(4)integrated over the azimuthal anglesφ1,φ2would be in principle sufficient [9].In this case the number of free parameters is reduced to4(the phases don’t enter) and only a3-dimensionalfit is required.We will see,however,that the information on these angles may substantially increase the precision of the P b determination.To simplify the error analysis,we follow ref.[9]and consider here only the most unfavourable situation,when the parameters P2b,|a+|2−|a−|2and|b+|2−|b−|2are much smaller thanα2Λ.In this case the moments<F i>can be considered to be independent, having the diagonal error matrixW=13,19,115,145,16135,16135,2135,2135,245,245).(7)Here N is a number of B b events(assuming that the background can be neglected,see next section).The error matrix V of the vector a of the parameters a j,j=1,..7defined in (6)isV( a)=(A T W−1A)−1,(8) where the elements of the matrix A are A ij=d(f1i.f2i)V11=δ0N,(9)δ0=1α2Λ.[(2r0−1)2180+4r2015+(1−r0)(1+coshχ)10.(10)Hereδ0depends only on the relative contribution r0of the decay amplitudes with helicityλ2=0and on the relative phaseχ(Figs.1a,b).The maximal error on P b is δmax=4.7Nand it corresponds to the case when r0=1√√√,Σ∗b →Λ0b πand the electromagnetic decays Ξ0′b →Ξ0b γor Ξ0∗b →Ξ0b γ.The observable polarization P obs depends on the polarizations P B b of direct beauty baryons and their production fractions b B b (i.e.probabilities of the b-quark to hadronize to certain baryons B b ).In considered decays the beauty baryon Λ0b or Ξ0b retains −13)of the polarizationof a parent with spin 12+)(see Appendix).For P obs we get:P obs =b Λ0b P Λ0b + i (−13b Σ∗bi P Σ∗bi )3b Ξ0′b P Ξ0′b+1b Ξ0b +b Ξ0′b +b Ξ0∗b.(12)The summation goes over positive,negative and neutral Σb and Σ∗b .Assuming the polar-ization of the heavier states to be similar in magnitude to that of directly produced Λ0b or Ξ0b (P Λ0b or P Ξ0b )we may expect the observed polarization in an interval of (0.34−0.67)P Λ0bfor Λ0b and (0.69−0.84)P Ξ0b for Ξ0b.The polarization can be measured for Λ0b and Ξ0b baryons and for their antiparticles.Λ0b (Ξ0b )are unambigously distinquishable from their antiparticles by effective mass of pπ−system from Λ0→pπ−decay.The wrong assignment of antiproton and pion masses gives the kinematical reflection ofΞ0b is governed by b→dcc.HoweverΛ0fromΞ0b→Ξ0J/ψorΞ−b→Ξ−J/ψis produced in a weak hyperon decay,so this background can be efficiently reduced by the cut on the minimal distance d between J/ψandΛ0.A conservative cut d<1.5mm reduces this background by a factor≈0.05(Fig.3b).The background from B0d→J/ψK0when one ofπmesons is considered as a proton is negligible after the effective mass cuts on(pπ)and(pπJ/ψ)systems.Background from fake J/ψ′s,as it has been shown in[18],can be reduced to a low level by cuts on the distance between the primary vertex and the production point of the J/ψcandidate and the distance of closest approach between the two particles from the decay.These cuts also suppress the background from real J/ψ′s comming directly from hadronization.The number of producedΛ0b andΞ0b is calculated for the cross section of pp→busing the last segment of the hadron calorimeter by its minimum ionizing signature.-ForΛ0J/ψ→pπ−e+e−decay both electrons are required to have p e⊥>1GeV.The low threshold for electrons is possible,because of electron identification in the transition radiation tracker(TRT)[24].The events are required to contain one muon with a pµ⊥> 6GeV and|η|<1.6The second set of cuts corresponds to’offline’analysis cuts.The same cuts as for B0d→J/ψK0reconstruction[18]can be used(the only exception is the mass requirement forΛ0candidate,see the last of the next cuts):-The two charged hadrons fromΛ0decay are required to be within the tracking volume |η|<2.5,and transverse momenta of both to be greater than0.5GeV.-Λ0decay length in the transverse plane with respect to the beam axis was required to be greater than1cm and less than50cm.The upper limit ensures that the charged tracks fromΛ0decay start before the inner radius of TRT,and that there is a space point from the innermost layer of the outer silicon tracker.The lower limit reduces the combinatorial background from particles originating from the primary vertex.-The distance of closest approach between the two muon(electron)candidates forming the J/ψwas required to be less than320µm(450µm),giving an acceptance for signal of 0.94.-The proper time of theΛ0b decay,measured from the distance between the primary vertex and the production point of the J/ψin the transverse plane and the reconstructed p⊥ofΛ0b,was required to be greater than0.5ps.This cut is used to reduce the combina-torial background,giving the acceptance for signal events0.68.-The reconstructedΛ0and J/ψmasses were required to be within two standart de-viations of nominal values.The results on expectedΛ0b andΞ0b statistics and the errors of their polarization mea-surement are summarized in Table2.For both channels the statistics of reconstructed events at the luminosity104pb−1will be790000(220000)Λ0b and2600(720)Ξ0b,where the values are derived using UA1(CDF)results.For this statistics the maximal value of the statistical error on the polarization mea-surement,calculated from formulae(9)and(10),will be0.005(0.01)forΛ0b and0.09(0.17) forΞ0b.7ConclusionAt LHC luminosity104pb−1the beauty baryonsΛ0b andΞ0b polarizations can be measured with the help of angular distributions in the cascade decaysΛ0J/ψ→pπ−µ+µ−and Λ0J/ψ→pπ−e+e−with the statistical precision better than0.010forΛ0b and0.17for Ξ0b.AppendixThe polarization transfered toΛ0b,which was produced indirectly in strongΣb andΣ∗b decays,depends on the ratio∆| p inc× pΣb|,where p inc and pΣb are momenta of incident particle andΣb in c.m.system.Ω1=(θ1,φ1)are the polar and the azimuthal angles ofΛ0inΛ0b rest frame with the axes defined as z1↑↑ pΛ0b,y1↑↑ n× pΛ0b.After the transformation ofΩ1→Ω′1ofΛ0angles from the helicity frame x1,y1,z1to the canonical frame x,y,z with z↑↑ n and the integration over cosθandφ′1we get the distribution of the cosine of the angle between theΛ0momentum vector(Λ0b decay analyzer)and theΣb orΣ∗b production normal(which can be considered coinciding with theΛ0b production normal due to a small energy release in theΣb orΣ∗b decays):w(cosθ′1)∼1∓13(13(1References[1]K.Heller,Proceedings of the VII-th Int.Symp.on High Energy SpinPhysics,Protvino,1986vol.I,p.81.[2]L.Pondrom,Phys.Rep.122(19985)57.[3]B.Andersson et al.,Phys.Lett.85B(1979)417.[4]T.A.De Grand,H.I.Miettinen,Phys.Rev.D24(1981)2419.[5]B.V.Struminsky,Yad.Fiz.34(1981)1954.[6]R.Lednicky,Czech.J.Phys.B33(1983)1177;Z.Phys.C26(1985)531.[7]P.M.Ho et al.,Phys.Rev.Lett.65(1990)1713.[8]A.Morelos et al.,FERMILAB-Pub-93/167-E.[9]R.Lednicky,Yad.Fiz.43(1986)1275(Sov.J.Nucl.Phys.43(1986),817).[10]G.Kane,Y.P.Yao,Nucl.Phys.B137(1978)313.[11]J.Szwed,Phys.Lett.105B(1981)403.[12]W.G.D.Dharmaratna,Gary R.Goldstein,Phys.Rev.D41(1990)1731.[13]B.V.Batyunya et al.,Czech.J.Phys.B31(1981)11.[14]C.M.Troshin,H.E.Tyurin,Yad.Fiz.38(1983)1065.[15]A.V.Efremov,O.V.Teryaev,Phys.Lett.B150(1985)383.[16]A.N.Aleev et al.,Yad.Fiz.43(1986)619.[17]P.Chauvatet et al.,Phys.Lett.199B(1987)304.[18]The ATLAS Collaboration,CERN/LHCC/93-53,Oct.1993.[19]W.Hoffmann,DESY93-026(1993).[20]H.Albrecht et al.,DESY93-156(1993).9[21]A.H.Ball et al.,J.Phys.G:Nucl.Part.Phys.18(1992)1703.[22]UA1Collaboration,Phys.Lett.273B(1991)544.[23]CDF Collaboration,Phys.Rev.D47(1993)R2639.[24]I.Gavrilenko,ATLAS Internal Note INDET-NO-016,1992.[25]A.F.Falk and M.E.Peskin,SLAC-PUB-6311,1993.[26]R.Lednicky,DrSc Thesis,JINR-Dubna1990,p.174(in russian).10i f 2i011P ba +a ∗+−a −a ∗−−b +b ∗++b −b ∗−cos θ13P b αΛ−a +a ∗+−a −a ∗−+12b −b ∗−d 200(θ2)52b +b ∗+−1P b −a +a ∗++a −a ∗−−12b −b ∗−d 200(θ2)cos θ172b +b ∗+−1P b αΛ8P b αΛ3Im (a +a ∗−)sin θsin θ1sin 2θ2sin φ1102Re (b −b ∗+)sin θsin θ1sin 2θ2cos (φ1+2φ2)112Im (b −b ∗+)sin θsin θ1sin 2θ2sin (φ1+2φ2)−32Re (b −a ∗++a −b ∗+)sin θcos θ1sin θ2cos θ2cos φ213√P b αΛ−32Re (b −a ∗−+a +b ∗+)cos θsin θ1sin θ2cos θ2cos(φ1+φ2)15√P b αΛ16√P b−32Im (a −b ∗+−b −a ∗+)sin θsin θ2cos θ2sin φ218√αΛ−32Im (b −a ∗−−a +b ∗+)sin θ1sin θ2cos θ2sin(φ1+φ2)Table 1:The coefficients f 1i ,f 2i and angular functions F i in distribution (4).11Parameter Value forΛ0b CommentL[cm−2s−1]1033b(b→B b)0.08br(B b→Λ0J/ψ)2.210−2(0.610−2)J/ψ→µ+µ−0.06Λ0→pπ−0.641.110−4(0.310−3)0.060.64b)500µbN(µ+µ−pπ−)accepted1535000pµ⊥>6GeV,|η|<1.6(426000)pµ⊥>3GeV,|η|<2.5pπ,p⊥>0.5GeV,|η|<2.5740(210)2400(670)N(µeepπ−)reconstructed65000(18000)the maximum statistical error0.005on the polarization measurement(0.010)δ(P b)Table2:Summary on beauty baryon measurement possibilities for LHC experiment AT-LAS.The values in brackets correspond to the CDF result,while the analogical values without brackets to the UA1result.12Figure1:The maximal statistical error on the polarization measurementδ(P b)andδ0=N+1Figure 2:The Λ0J/ψeffective mass distribution:The peak at 5.62GeV is from Λ0b and background comes from J/ψfrom a b-hadron decay and Λ0either from the multiparticle production or from a b-hadron decay (a).The events that passed the cut on the transverse momenta (p T >0.5GeV )for p and π−from Λ0decay (b).14Figure 3:The Λ0J/ψeffective mass distribution:The peak at 5.84GeV is from Ξ0b →Λ0J/ψdecay.The background with the centre at ≈5.5GeV comes from Ξ0b →Ξ0J/ψ,Ξ0→Λ0π0and Ξ−b →Ξ−J/ψ,Ξ−→Λ0π−decays (a).The events that passed the cut on the minimal distance of J/ψand Λ0(d <1.5mm )(b).15。
Measurement of the Branching Fractions and CP Asymmetries of B--D0(cp)K- Decays with the Ba

a r X i v :h e p -e x /0408082v 1 17 A u g 2004B A B A R -CONF-04/039SLAC-PUB-10655Measurement of the Branching Fractions and CP Asymmetries of B −→D 0(CP )K −Decays with the B A B A R Detector The B A B A R Collaboration February 7,2008Abstract We present a study of B −→D 0(CP )K −decays,where D 0(CP )is reconstructed in flavor (K −π+),CP -even (K −K +,π−π+)and CP -odd (K 0S π0)eigenstates,based on a sample of about 214million Υ(4S )→BB (B −→D 0K −)/B (B −→D 0π−)=0.87±0.14(stat)±0.06(syst),R −≡B (B −→D 0CP −K −)/B (B −→D 0CP −π−)B (B −→D 0CP +K −)+B (B +→D 0CP +K +)=0.40±0.15(stat)±0.08(syst)B(B−→D0CP−K−)−B(B+→D0CP−K+)A CP−≡Work supported in part by Department of Energy contract DE-AC03-76SF00515.The B A B A R Collaboration,B.Aubert,R.Barate,D.Boutigny,F.Couderc,J.-M.Gaillard,A.Hicheur,Y.Karyotakis,J.P.Lees,V.Tisserand,A.ZghicheLaboratoire de Physique des Particules,F-74941Annecy-le-Vieux,FranceA.Palano,A.PompiliUniversit`a di Bari,Dipartimento di Fisica and INFN,I-70126Bari,ItalyJ.C.Chen,N.D.Qi,G.Rong,P.Wang,Y.S.ZhuInstitute of High Energy Physics,Beijing100039,ChinaG.Eigen,I.Ofte,B.StuguUniversity of Bergen,Inst.of Physics,N-5007Bergen,NorwayG.S.Abrams,A.W.Borgland,A.B.Breon,D.N.Brown,J.Button-Shafer,R.N.Cahn,E.Charles, C.T.Day,M.S.Gill,A.V.Gritsan,Y.Groysman,R.G.Jacobsen,R.W.Kadel,J.Kadyk,L.T.Kerth,Yu.G.Kolomensky,G.Kukartsev,G.Lynch,L.M.Mir,P.J.Oddone,T.J.Orimoto,M.Pripstein,N.A.Roe,M.T.Ronan,V.G.Shelkov,W.A.WenzelLawrence Berkeley National Laboratory and University of California,Berkeley,CA94720,USAM.Barrett,K.E.Ford,T.J.Harrison,A.J.Hart,C.M.Hawkes,S.E.Morgan,A.T.Watson University of Birmingham,Birmingham,B152TT,United KingdomM.Fritsch,K.Goetzen,T.Held,H.Koch,B.Lewandowski,M.Pelizaeus,M.SteinkeRuhr Universit¨a t Bochum,Institut f¨u r Experimentalphysik1,D-44780Bochum,GermanyJ.T.Boyd,N.Chevalier,W.N.Cottingham,M.P.Kelly,tham,F.F.WilsonUniversity of Bristol,Bristol BS81TL,United KingdomT.Cuhadar-Donszelmann,C.Hearty,N.S.Knecht,T.S.Mattison,J.A.McKenna,D.Thiessen University of British Columbia,Vancouver,BC,Canada V6T1Z1A.Khan,P.Kyberd,L.TeodorescuBrunel University,Uxbridge,Middlesex UB83PH,United KingdomA.E.Blinov,V.E.Blinov,V.P.Druzhinin,V.B.Golubev,V.N.Ivanchenko,E.A.Kravchenko,A.P.Onuchin,S.I.Serednyakov,Yu.I.Skovpen,E.P.Solodov,A.N.YushkovBudker Institute of Nuclear Physics,Novosibirsk630090,RussiaD.Best,M.Bruinsma,M.Chao,I.Eschrich,D.Kirkby,nkford,M.Mandelkern,R.K.Mommsen,W.Roethel,D.P.StokerUniversity of California at Irvine,Irvine,CA92697,USAC.Buchanan,B.L.HartfielUniversity of California at Los Angeles,Los Angeles,CA90024,USAS.D.Foulkes,J.W.Gary,B.C.Shen,K.WangUniversity of California at Riverside,Riverside,CA92521,USAD.del Re,H.K.Hadavand,E.J.Hill,D.B.MacFarlane,H.P.Paar,Sh.Rahatlou,V.SharmaUniversity of California at San Diego,La Jolla,CA92093,USAJ.W.Berryhill,C.Campagnari,B.Dahmes,O.Long,A.Lu,M.A.Mazur,J.D.Richman,W.Verkerke University of California at Santa Barbara,Santa Barbara,CA93106,USAT.W.Beck,A.M.Eisner,C.A.Heusch,J.Kroseberg,W.S.Lockman,G.Nesom,T.Schalk,B.A.Schumm,A.Seiden,P.Spradlin,D.C.Williams,M.G.WilsonUniversity of California at Santa Cruz,Institute for Particle Physics,Santa Cruz,CA95064,USAJ.Albert,E.Chen,G.P.Dubois-Felsmann,A.Dvoretskii,D.G.Hitlin,I.Narsky,T.Piatenko,F.C.Porter,A.Ryd,A.Samuel,S.YangCalifornia Institute of Technology,Pasadena,CA91125,USAS.Jayatilleke,G.Mancinelli,B.T.Meadows,M.D.SokoloffUniversity of Cincinnati,Cincinnati,OH45221,USAT.Abe,F.Blanc,P.Bloom,S.Chen,W.T.Ford,U.Nauenberg,A.Olivas,P.Rankin,J.G.Smith,J.Zhang,L.ZhangUniversity of Colorado,Boulder,CO80309,USAA.Chen,J.L.Harton,A.Soffer,W.H.Toki,R.J.Wilson,Q.ZengColorado State University,Fort Collins,CO80523,USAD.Altenburg,T.Brandt,J.Brose,M.Dickopp,E.Feltresi,A.Hauke,cker,R.M¨u ller-Pfefferkorn, R.Nogowski,S.Otto,A.Petzold,J.Schubert,K.R.Schubert,R.Schwierz,B.Spaan,J.E.Sundermann Technische Universit¨a t Dresden,Institut f¨u r Kern-und Teilchenphysik,D-01062Dresden,GermanyD.Bernard,G.R.Bonneaud,F.Brochard,P.Grenier,S.Schrenk,Ch.Thiebaux,G.Vasileiadis,M.VerderiEcole Polytechnique,LLR,F-91128Palaiseau,FranceD.J.Bard,P.J.Clark,vin,F.Muheim,S.Playfer,Y.XieUniversity of Edinburgh,Edinburgh EH93JZ,United KingdomM.Andreotti,V.Azzolini,D.Bettoni,C.Bozzi,R.Calabrese,G.Cibinetto,E.Luppi,M.Negrini,L.Piemontese,A.SartiUniversit`a di Ferrara,Dipartimento di Fisica and INFN,I-44100Ferrara,ItalyE.TreadwellFlorida A&M University,Tallahassee,FL32307,USAF.Anulli,R.Baldini-Ferroli,A.Calcaterra,R.de Sangro,G.Finocchiaro,P.Patteri,I.M.Peruzzi,M.Piccolo,A.ZalloLaboratori Nazionali di Frascati dell’INFN,I-00044Frascati,ItalyA.Buzzo,R.Capra,R.Contri,G.Crosetti,M.Lo Vetere,M.Macri,M.R.Monge,S.Passaggio,C.Patrignani,E.Robutti,A.Santroni,S.TosiUniversit`a di Genova,Dipartimento di Fisica and INFN,I-16146Genova,ItalyS.Bailey,G.Brandenburg,K.S.Chaisanguanthum,M.Morii,E.WonHarvard University,Cambridge,MA02138,USAR.S.Dubitzky,ngeneggerUniversit¨a t Heidelberg,Physikalisches Institut,Philosophenweg12,D-69120Heidelberg,Germany W.Bhimji,D.A.Bowerman,P.D.Dauncey,U.Egede,J.R.Gaillard,G.W.Morton,J.A.Nash,M.B.Nikolich,G.P.TaylorImperial College London,London,SW72AZ,United KingdomM.J.Charles,G.J.Grenier,U.MallikUniversity of Iowa,Iowa City,IA52242,USAJ.Cochran,H.B.Crawley,msa,W.T.Meyer,S.Prell,E.I.Rosenberg,A.E.Rubin,J.YiIowa State University,Ames,IA50011-3160,USAM.Biasini,R.Covarelli,M.PioppiUniversit`a di Perugia,Dipartimento di Fisica and INFN,I-06100Perugia,ItalyM.Davier,X.Giroux,G.Grosdidier,A.H¨o cker,place,F.Le Diberder,V.Lepeltier,A.M.Lutz, T.C.Petersen,S.Plaszczynski,M.H.Schune,L.Tantot,G.WormserLaboratoire de l’Acc´e l´e rateur Lin´e aire,F-91898Orsay,FranceC.H.Cheng,nge,M.C.Simani,D.M.WrightLawrence Livermore National Laboratory,Livermore,CA94550,USAA.J.Bevan,C.A.Chavez,J.P.Coleman,I.J.Forster,J.R.Fry,E.Gabathuler,R.Gamet,D.E.Hutchcroft,R.J.Parry,D.J.Payne,R.J.Sloane,C.TouramanisUniversity of Liverpool,Liverpool L6972E,United KingdomJ.J.Back,1C.M.Cormack,P.F.Harrison,1F.Di Lodovico,G.B.Mohanty1Queen Mary,University of London,E14NS,United KingdomC.L.Brown,G.Cowan,R.L.Flack,H.U.Flaecher,M.G.Green,P.S.Jackson,T.R.McMahon,S.Ricciardi,F.Salvatore,M.A.WinterUniversity of London,Royal Holloway and Bedford New College,Egham,Surrey TW200EX,United KingdomD.Brown,C.L.DavisUniversity of Louisville,Louisville,KY40292,USAJ.Allison,N.R.Barlow,R.J.Barlow,P.A.Hart,M.C.Hodgkinson,fferty,A.J.Lyon,J.C.WilliamsUniversity of Manchester,Manchester M139PL,United KingdomA.Farbin,W.D.Hulsbergen,A.Jawahery,D.Kovalskyi,e,V.Lillard,D.A.RobertsUniversity of Maryland,College Park,MD20742,USAG.Blaylock,C.Dallapiccola,K.T.Flood,S.S.Hertzbach,R.Kofler,V.B.Koptchev,T.B.Moore,S.Saremi,H.Staengle,S.WillocqUniversity of Massachusetts,Amherst,MA01003,USAR.Cowan,G.Sciolla,S.J.Sekula,F.Taylor,R.K.Yamamoto Massachusetts Institute of Technology,Laboratory for Nuclear Science,Cambridge,MA02139,USAD.J.J.Mangeol,P.M.Patel,S.H.RobertsonMcGill University,Montr´e al,QC,Canada H3A2T8zzaro,V.Lombardo,F.PalomboUniversit`a di Milano,Dipartimento di Fisica and INFN,I-20133Milano,ItalyJ.M.Bauer,L.Cremaldi,V.Eschenburg,R.Godang,R.Kroeger,J.Reidy,D.A.Sanders,D.J.Summers,H.W.ZhaoUniversity of Mississippi,University,MS38677,USAS.Brunet,D.Cˆo t´e,P.TarasUniversit´e de Montr´e al,Laboratoire Ren´e J.A.L´e vesque,Montr´e al,QC,Canada H3C3J7H.NicholsonMount Holyoke College,South Hadley,MA01075,USAN.Cavallo,2F.Fabozzi,2C.Gatto,L.Lista,D.Monorchio,P.Paolucci,D.Piccolo,C.Sciacca Universit`a di Napoli Federico II,Dipartimento di Scienze Fisiche and INFN,I-80126,Napoli,ItalyM.Baak,H.Bulten,G.Raven,H.L.Snoek,L.WildenNIKHEF,National Institute for Nuclear Physics and High Energy Physics,NL-1009DB Amsterdam,The NetherlandsC.P.Jessop,J.M.LoSeccoUniversity of Notre Dame,Notre Dame,IN46556,USAT.Allmendinger,K.K.Gan,K.Honscheid,D.Hufnagel,H.Kagan,R.Kass,T.Pulliam,A.M.Rahimi,R.Ter-Antonyan,Q.K.WongOhio State University,Columbus,OH43210,USAJ.Brau,R.Frey,O.Igonkina,C.T.Potter,N.B.Sinev,D.Strom,E.TorrenceUniversity of Oregon,Eugene,OR97403,USAF.Colecchia,A.Dorigo,F.Galeazzi,M.Margoni,M.Morandin,M.Posocco,M.Rotondo,F.Simonetto,R.Stroili,G.Tiozzo,C.VociUniversit`a di Padova,Dipartimento di Fisica and INFN,I-35131Padova,ItalyM.Benayoun,H.Briand,J.Chauveau,P.David,Ch.de la Vaissi`e re,L.Del Buono,O.Hamon,M.J.J.John,Ph.Leruste,J.Malcles,J.Ocariz,M.Pivk,L.Roos,S.T’Jampens,G.Therin Universit´e s Paris VI et VII,Laboratoire de Physique Nucl´e aire et de Hautes Energies,F-75252Paris,FranceP.F.Manfredi,V.ReUniversit`a di Pavia,Dipartimento di Elettronica and INFN,I-27100Pavia,ItalyP.K.Behera,L.Gladney,Q.H.Guo,J.PanettaUniversity of Pennsylvania,Philadelphia,PA19104,USAC.Angelini,G.Batignani,S.Bettarini,M.Bondioli,F.Bucci,G.Calderini,M.Carpinelli,F.Forti, M.A.Giorgi,A.Lusiani,G.Marchiori,F.Martinez-Vidal,3M.Morganti,N.Neri,E.Paoloni,M.Rama,G.Rizzo,F.Sandrelli,J.WalshUniversit`a di Pisa,Dipartimento di Fisica,Scuola Normale Superiore and INFN,I-56127Pisa,ItalyM.Haire,D.Judd,K.Paick,D.E.WagonerPrairie View A&M University,Prairie View,TX77446,USAN.Danielson,P.Elmer,u,C.Lu,V.Miftakov,J.Olsen,A.J.S.Smith,A.V.TelnovPrinceton University,Princeton,NJ08544,USAF.Bellini,G.Cavoto,4R.Faccini,F.Ferrarotto,F.Ferroni,M.Gaspero,L.Li Gioi,M.A.Mazzoni,S.Morganti,M.Pierini,G.Piredda,F.Safai Tehrani,C.VoenaUniversit`a di Roma La Sapienza,Dipartimento di Fisica and INFN,I-00185Roma,ItalyS.Christ,G.Wagner,R.WaldiUniversit¨a t Rostock,D-18051Rostock,GermanyT.Adye,N.De Groot,B.Franek,N.I.Geddes,G.P.Gopal,E.O.Olaiya Rutherford Appleton Laboratory,Chilton,Didcot,Oxon,OX110QX,United KingdomR.Aleksan,S.Emery,A.Gaidot,S.F.Ganzhur,P.-F.Giraud,G.Hamel de Monchenault,W.Kozanecki, M.Legendre,G.W.London,B.Mayer,G.Schott,G.Vasseur,Ch.Y`e che,M.ZitoDSM/Dapnia,CEA/Saclay,F-91191Gif-sur-Yvette,FranceM.V.Purohit,A.W.Weidemann,J.R.Wilson,F.X.YumicevaUniversity of South Carolina,Columbia,SC29208,USAD.Aston,R.Bartoldus,N.Berger,A.M.Boyarski,O.L.Buchmueller,R.Claus,M.R.Convery,M.Cristinziani,G.De Nardo,D.Dong,J.Dorfan,D.Dujmic,W.Dunwoodie,E.E.Elsen,S.Fan, R.C.Field,T.Glanzman,S.J.Gowdy,T.Hadig,V.Halyo,C.Hast,T.Hryn’ova,W.R.Innes, M.H.Kelsey,P.Kim,M.L.Kocian,D.W.G.S.Leith,J.Libby,S.Luitz,V.Luth,H.L.Lynch,H.Marsiske,R.Messner,D.R.Muller,C.P.O’Grady,V.E.Ozcan,A.Perazzo,M.Perl,S.Petrak, B.N.Ratcliff,A.Roodman,A.A.Salnikov,R.H.Schindler,J.Schwiening,G.Simi,A.Snyder,A.Soha,J.Stelzer,D.Su,M.K.Sullivan,J.Va’vra,S.R.Wagner,M.Weaver,A.J.R.Weinstein, W.J.Wisniewski,M.Wittgen,D.H.Wright,A.K.Yarritu,C.C.YoungStanford Linear Accelerator Center,Stanford,CA94309,USAP.R.Burchat,A.J.Edwards,T.I.Meyer,B.A.Petersen,C.RoatStanford University,Stanford,CA94305-4060,USAS.Ahmed,M.S.Alam,J.A.Ernst,M.A.Saeed,M.Saleem,F.R.WapplerState University of New York,Albany,NY12222,USAW.Bugg,M.Krishnamurthy,S.M.SpanierUniversity of Tennessee,Knoxville,TN37996,USAR.Eckmann,H.Kim,J.L.Ritchie,A.Satpathy,R.F.SchwittersUniversity of Texas at Austin,Austin,TX78712,USAJ.M.Izen,I.Kitayama,X.C.Lou,S.YeUniversity of Texas at Dallas,Richardson,TX75083,USAF.Bianchi,M.Bona,F.Gallo,D.GambaUniversit`a di Torino,Dipartimento di Fisica Sperimentale and INFN,I-10125Torino,ItalyL.Bosisio,C.Cartaro,F.Cossutti,G.Della Ricca,S.Dittongo,S.Grancagnolo,nceri,P.Poropat,5L.Vitale,G.VuagninUniversit`a di Trieste,Dipartimento di Fisica and INFN,I-34127Trieste,ItalyR.S.PanviniVanderbilt University,Nashville,TN37235,USASw.Banerjee,C.M.Brown,D.Fortin,P.D.Jackson,R.Kowalewski,J.M.Roney,R.J.SobieUniversity of Victoria,Victoria,BC,Canada V8W3P6H.R.Band,B.Cheng,S.Dasu,M.Datta,A.M.Eichenbaum,M.Graham,J.J.Hollar,J.R.Johnson,P.E.Kutter,H.Li,R.Liu,A.Mihalyi,A.K.Mohapatra,Y.Pan,R.Prepost,P.Tan,J.H.vonWimmersperg-Toeller,J.Wu,S.L.Wu,Z.YuUniversity of Wisconsin,Madison,WI53706,USAM.G.Greene,H.NealYale University,New Haven,CT06511,USA1INTRODUCTIONA theoretically clean measurement of the angleγ=arg(−V ud V∗ub/V cd V∗cb)can be obtained from the study of B−→D(∗)0K(∗)−decays by exploiting the interference between the b→c¯u s and b→u¯c s decay amplitudes[1].The method originally proposed by Gronau,Wyler and London is based on the interference between B−→D0K−and B−→D0decay to CP eigenstates.We define the ratios R and R CP±of Cabibbo-suppressed to Cabibbo-favored branching fractionsR(CP±)≡B(B−→D0(CP±)K−)+B(B+→B(B−→D0(CP±)π−)+B(B+→B(B−→D0CP±K−)+B(B+→D0CP±K+).(2)Neglecting the D0−D0π−)/A(B−→D0π−)of the amplitudes of the B−→D0K−)/A(B−→D0K−)|is the magnitude of the ratio of the amplitudes for the processes B−→B pairs collected with the B A B A R detector at the PEP-II asymmetric-energy B factory.The B A B A R detector is described in detail elsewhere[2].Charged-particle tracking is provided by afive-layer silicon vertex tracker(SVT)and a40-layer drift chamber(DCH).For charged-particle identification,ionization energy loss in the DCH and SVT,and Cherenkov radia-tion detected in a ring-imaging device(DIRC)are used.Photons are identified by the electromag-netic calorimeter(EMC),which comprises6580thallium-doped CsI crystals.These systems are mounted inside a1.5-T solenoidal superconducting magnet.The segmentedflux return,including endcaps,is instrumented with resistive plate chambers(IFR)for muon and K0Lidentification.We use the GEANT[3]software to simulate interactions of particles traversing the detector,taking into account the varying accelerator and detector conditions.3ANALYSIS METHODWe reconstruct B−→D0h−decays,where the prompt track h−is a kaon or a pion.Reference to the charge-conjugate state is implied here and throughout the text unless otherwise stated.Candidates for D0are reconstructed in the CP-even eigenstatesπ−π+and K−K+,in the CP-odd eigenstate K0Sπ0,and in the non-CPflavor eigenstate K−π+.K0Scandidates are selected in theπ−π+channel.The prompt particle h−is required to have momentum greater than1.4GeV/c.Particle IDinformation from the drift chamber and,when available,from the DIRC must be consistent with the kaon hypothesis for the K meson candidate in all D0modes and with the pion hypothesis for theπ±meson candidates in the D0→π−π+mode.For the prompt track to be identified as a pion or a kaon,we require that at leastfive Cherenkov photons are detected to insure a good measurement of the Cherenkov angle.We reject a candidate track if its Cherenkov angle is not within3σof the expected value for either the kaon or pion mass hypothesis.We also reject candidate tracks that are identified as electrons by the DCH and the EMC or as muons by the DCH and the IFR.Photon candidates are clusters in the EMC that are not matched to any charged track,have a raw energy greater than30MeV and lateral shower shape consistent with the expected pattern of energy deposit from an electromagnetic shower.Photon pairs with invariant mass within the range 115–150MeV/c2(∼3σ)and total energy greater than200MeV are consideredπ0candidates. To improve the momentum resolution,theπ0candidates are kinematicallyfit with their mass constrained to the nominalπ0mass[4].Neutral kaons are reconstructed from pairs of oppositely charged tracks with the invariant mass within10MeV(∼3σ)from the nominal K0mass.We also require that the ratio between theflight length distance in the plane transverse to the beams direction and its uncertainty is greater than 3.The invariant mass of a D0candidate,m(D0),must be within3σof the D0mass.The D0mass resolutionσis about7.5MeV in the K−π+,K−K+andπ−π+modes,and about21MeV in the π0mode.Selected D0candidates arefitted with a constraint to the nominal D0mass.K0SWe reconstruct B meson candidates by combining a D0candidate with a track h−.For the K−π+mode,the charge of the track h−must match that of the kaon from the D0me-son decay.We select B meson candidates by using the beam-energy-substituted mass m ES=rejects more than90%of the continuum background while retaining77%of the signal in the K−π+, K−K+and K0π0modes and65%in theπ−π+channel.SMultiple B−→D0h−candidates are found in about4%of the events for the K0Sπ0and in less than1%of the events for the other D0decays.In these events aχ2is constructed from m(π0)(for K0Sπ0only),m(D0),and m ES and only the candidate with the smallestχ2is retained.The total reconstruction efficiencies,based on simulated signal events,are about33%(K−π+),28%(K−K+), 26%(π−π+)and17%(K0Sπ0).The main contributions to the BB events,in which the prompt track is either a pion or a kaon.The input variables to thefit are∆E and a particle identification probability for the prompt track based on the Cherenkov angleθC,the momentum p and the polar angleθof the track.The extended likelihood function L is defined asL=exp −M i=1n i N j=1 M i=1n i P i(∆E,θC; αi) ,(3)where N is the total number of observed events.The M functions P i(∆E,θC; αi)are the probability density functions(PDFs)for the variables∆E,θC,given the set of parameters αi.Since these two quantities are sufficiently uncorrelated,their probability density functions are evaluated as a product P i=P i(∆E; αi)×P i(θC; αi).The∆E distribution for B−→D0K−signal events is parametrized with a Gaussian function. The∆E distribution for B−→D0π−is parametrized with the same Gaussian used for B−→D0K−with a relative shift of the mean,computed event by event as a function of the prompt track momentum,arising from the wrong mass assignment to the prompt track.The offset and width of the Gaussian are keptfloating in thefit and are determined from data together with the yields.The∆E distribution for the continuum background is parametrized with a linear function whose slope is determined from off-resonance data.The∆E distribution for the B4PHYSICS RESULTS AND SYSTEMATIC STUDIESThe results of thefit are summarized in Table1.Figure1shows the distributions of∆E for the K−π+,CP+and CP−modes after enhancing the B→D0K purity by requiring that the prompt track be consistent with the kaon hypothesis.This requirement is about95%efficient for the B−→D0K−signal while retaining only4%of the B−→D0π−candidates.The projection of a likelihoodfit,modified to take into account the tighter selection criteria,is overlaid in thefigure. Table1:Results of the B−→D0K−and B−→D0π−yields from the maximum-likelihoodfit on data.D0mode N(B→D0π)N(B→D0K)N(B−→D0K−)N(B+→K−π+11930±120897±34441±24456±25K0Sπ01248±4076+13−1246+10−930+9−8The double ratios R±are computed by scaling the ratios of the numbers of B−→D0K−and B−→D0π−mesons by correction factors(ranging from0.997to1.020depending on the D0mode) that account for small differences in the efficiency between the B−→D0K−and B−→D0π−selec-tions,estimated with simulated signal samples.The results are listed in Table2.The direct CP asymmetries A CP±for the B±→D0CP±K±decays are calculated from the measured yields of positive and negative charged meson decays and the results are reported in Table2.Table2:Measured double branching fraction ratios R±and CP asymmetries A CP±for different D0decay modes.Thefirst error is statistical,the second is systematic.D0decay mode R CP/R A CPK−K+0.92±0.16±0.070.43±0.16±0.09π−π+0.70±0.29±0.090.27±0.40±0.09CP-even combined0.87±0.14±0.060.40±0.15±0.08The uncertainties in the branching fractions of the channels contributing to the B[6]Belle Collaboration,K.Abe et al.,Phys.Rev.D6*******(2002);B A B A R Collaboration,B.Aubert et al.,hep-ex/0308065,submitted to Phys.Rev.Lett..[7]B A B A R Collaboration,B.Aubert et al.,Phys.Rev.Lett.92202002(2004).Figure1:∆E distributions of B−→D0h−candidates,where a charged kaon mass hypothesis is assumed for h.Events are enhanced in B−→D0K−purity by requiring the Cherenkov angle of the track h to be within2σof the kaon hypothesis.Top:B−→D0[K−π+]K−;middle:B−→π0]K−.Solid curves represent projections of D0CP+[K−K+,π−π+]K−;bottom:B−→D0CP−[K0Sthe maximum likelihoodfit;dashed-dotted,dotted and dashed curves represent the B→D0K,B→D0πand background contributions.。
2022年考研考博-考博英语-中国人民大学考试全真模拟易错、难点剖析AB卷(带答案)试题号:50

2022年考研考博-考博英语-中国人民大学考试全真模拟易错、难点剖析AB卷(带答案)一.综合题(共15题)1.单选题In most countries, the metric system has been ()for all measurement.问题1选项A.admittedB.adaptedC.appliedD.adopted【答案】D【解析】动词词义辨析。
句意:在大多数国家,所有的计量都采用公制。
选项D符合句意。
2.单选题In his typically American open style of communication, Mr. Hayes confronted Isabeta about not looking at him. Reluctantly, she explained why. As a newcomer from Mexico, she had been taught to avoid eye contact as a mark of respect to authority figures, teachers, employers, parents. Mr. Hayes did not know this. He then informed her that most Americans interpret lack of eye contact as disrespect and deviousness. Ultimately, he convinced Isabela to try and change her habit, which she slowly did.People from many Asian, Latin American, and Caribbean cultures also avoid eye contact as a sign of respect. Many African Americans, especially from the South, observe this custom, too.A master’s thesis by Samuel Avoian, a graduate student at Central Missouri State Univ ersity, tells how misinterpreting eye-contact customs can have a negative impact when white football coaches recruit African American players for the teams.He reports that, when speaking, white communicators usually look away from the listener, only periodically glancing at them. They do the opposite when listening. They are expected to look at the speaker all the time. Many African Americans communicate in an opposite way. When speaking, they tend to constantly stare at the listener; when listening, they mostly look away. Therefore, if white sports recruiters are not informed about these significant differences, they can be misled about interest and attentiveness when interviewing prospective African American ball players.In multicultural America, issues of eye contact have brought about social conflicts of two different kinds in many urban centers, non-Korean customers became angry when Korean shopkeepers did not look at them directly. The customers translated the lack of eye contact as a sign of disrespect, a habit blamed for contributing to the open confrontation taking place between some Asians and African Americans in New York, Texas, and California. Many teachers too have provided stories about classroom conflicts based on their misunderstanding Asian and Latin American children lack of eye contact as being disrespectful.On the other hand, direct eye contact has now taken on a new meaning among the younger generation and across ethnic borders. Particularly in urban centers, when one teenager looks directly at another, this is considered a provocation, sometimes called mad-dogging, and can lead to physical conflict.Mad-dogging has become the source of many campus conflicts. In one high school, it resultedin a fight between Cambodian newcomers and African-American students. The Cambodians had been staring at the other students merely to learn how Americans behave, yet the others misinterpreted the Cambodians’ intentions and the fight began.Mad-dogging seems to be connected with the avoidance of eye contact as a sign of respect. Thus, in the urban contemporary youth scene, if one looks directly at another, this disrespects, or “disses,” that person. Much like the archaic phrase “I demand satisfaction,” which became the overture to a duel. Mad-dogging may become a prelude to a physical encounter. At the entrances to Universal Studio’s “City Walk” attraction in Los Angeles, they have posted Code of Conduct signs. The second rule warns against “physically over bally threatening any person, fighting, annoying others through noisy or boisterous activities or by unnecessary staring...”1.Many African Americans from the South() .2.When listening to the others, white communicators tend to() .3.Many customers in American cities are angry with Korean shopkeepers because() .4.Mad-dogging refers to() .5.The archaic phrase, “I demand satisfaction”() .问题1选项A.adopt a typically American open style of communicationB.often misinterpret the meaning of eye contactC.avoid eye contact as a sign of respectD.are taught to avoid eye contact whenever telling to the others问题2选项A.look at the speaker all the timeB.glance at the speaker periodicallyC.look away from the speakerD.stare at the speaker问题3选项A.Korean shopkeepers do not look at them directlyB.they expect a more enthusiastic reflection from the shopkeepersC.there are some social conflicts in many urban centersD.they are not informed about difference between cultures问题4选项A.a provocation from one teenager to another of a different ethnic backgroundB.physical conflict among the younger generation in urban centersC.a lack of eye contact as a sign of respectD.the source of many campus conflicts across ethnic borders in urban centers问题5选项A.was connected with the avoidance of eye contactB.often led to a fightC.was a sign of disrespectD.often resulted in some kind of misinterpretation【答案】第1题:C第2题:A第3题:A第4题:A第5题:B【解析】1.根据关键词“African Americans, from the South”定位到第二段第二句“Many African Americans, especially from the Sout h, observe this custom, too.”这里的“custom”即上一句提到的“avoid eye contact as a sign of respect.”选项C与原文描述一致。
测控专业英语3

译为:测量是科学、工程、建筑及其他技术,甚至日常生活的 基础。
Measurement
(1) element In general, measuring systems comprise a number of functional elements. One element is required to discriminate the measurand and sense its dimensions or frequency. This information is then transmitted throughout the system by physical signals. If the measurand is itself active, such as water flow, it may power the signal; if passive, it must trigger the signal by interaction either with an energetic probe, such as a light source or X--ray tube, or with a carrier signal. Eventually the physical signal is compared with a reference signal of known quantity that has been subdivided or multiplied to suit the range of measurement required. The reference signal is derived from measurands of known quantity by a process called calibration.
Measurement of the Branching Fractions of $Lambda_{c}^{+}to pbar{K}n(pi)$

a rXiv:h ep-e x /97912v11Sep1997CLNS 97/1502CLEO 97-18Measurement of the Branching Fractions of Λ+c →p K 0,p K 0π0,all measured relative to pK −π+.The relative branching fractions are 0.67±0.04±0.11,0.46±0.02±0.04,0.52±0.04±0.05,and 0.66±0.05±0.07respectively.M.S.Alam,1S.B.Athar,1Z.Ling,1A.H.Mahmood,1H.Severini,1S.Timm,1F.Wappler,1A.Anastassov,2J.E.Duboscq,2D.Fujino,2,∗K.K.Gan,2T.Hart,2 K.Honscheid,2H.Kagan,2R.Kass,2J.Lee,2M.B.Spencer,2M.Sung,2A.Undrus,2,†R.Wanke,2A.Wolf,2M.M.Zoeller,2B.Nemati,3S.J.Richichi,3W.R.Ross,3P.Skubic,3 M.Bishai,4J.Fast,4J.W.Hinson,4N.Menon,ler,4E.I.Shibata,4I.P.J.Shipsey,4M.Yurko,4L.Gibbons,5S.Glenn,5S.D.Johnson,5Y.Kwon,5,‡S.Roberts,5E.H.Thorndike,5C.P.Jessop,6K.Lingel,6H.Marsiske,6M.L.Perl,6 D.Ugolini,6R.Wang,6X.Zhou,6T.E.Coan,7V.Fadeyev,7I.Korolkov,7Y.Maravin,7 I.Narsky,7V.Shelkov,7J.Staeck,7R.Stroynowski,7I.Volobouev,7J.Ye,7M.Artuso,8 A.Efimov,8M.Goldberg,8D.He,8S.Kopp,8G.C.Moneti,8R.Mountain,8S.Schuh,8 T.Skwarnicki,8S.Stone,8G.Viehhauser,8X.Xing,8J.Bartelt,9S.E.Csorna,9V.Jain,9,§K.W.McLean,9S.Marka,9R.Godang,10K.Kinoshita,i,10P.Pomianowski,10 S.Schrenk,10G.Bonvicini,11D.Cinabro,11R.Greene,11L.P.Perera,11G.J.Zhou,11B.Barish,12M.Chadha,12S.Chan,12G.Eigen,ler,12C.O’Grady,12M.Schmidtler,12J.Urheim,12A.J.Weinstein,12F.W¨u rthwein,12D.W.Bliss,13G.Masek,13H.P.Paar,13S.Prell,13V.Sharma,13D.M.Asner,14J.Gronberg,14T.S.Hill,nge,14S.Menary,14R.J.Morrison,14H.N.Nelson,14T.K.Nelson,14C.Qiao,14J.D.Richman,14D.Roberts,14A.Ryd,14M.S.Witherell,14R.Balest,15B.H.Behrens,15W.T.Ford,15H.Park,15J.Roy,15J.G.Smith,15J.P.Alexander,16C.Bebek,16B.E.Berger,16K.Berkelman,16K.Bloom,16D.G.Cassel,16H.A.Cho,16D.S.Crowcroft,16M.Dickson,16P.S.Drell,16K.M.Ecklund,16R.Ehrlich,16A.D.Foland,16P.Gaidarev,16B.Gittelman,16S.W.Gray,16D.L.Hartill,16B.K.Heltsley,16P.I.Hopman,16J.Kandaswamy,16P.C.Kim,16D.L.Kreinick,16 T.Lee,16Y.Liu,16G.S.Ludwig,16N.B.Mistry,16C.R.Ng,16E.Nordberg,16M.Ogg,16,∗∗J.R.Patterson,16D.Peterson,16D.Riley,16A.Soffer,16B.Valant-Spaight,16C.Ward,16 M.Athanas,17P.Avery,17C.D.Jones,17M.Lohner,17C.Prescott,17J.Yelton,17J.Zheng,17G.Brandenburg,18R.A.Briere,18A.Ershov,18Y.S.Gao,18D.Y.-J.Kim,18 R.Wilson,18H.Yamamoto,18T.E.Browder,19F.Li,19Y.Li,19J.L.Rodriguez,19T.Bergfeld,20B.I.Eisenstein,20J.Ernst,20G.E.Gladding,20G.D.Gollin,20 R.M.Hans,20E.Johnson,20I.Karliner,20M.A.Marsh,20M.Palmer,20M.Selen,20 J.J.Thaler,20K.W.Edwards,21A.Bellerive,22R.Janicek,22D.B.MacFarlane,22P.M.Patel,22A.J.Sadoff,23R.Ammar,24P.Baringer,24A.Bean,24D.Besson,24D.Coppage,24C.Darling,24R.Davis,24N.Hancock,24S.Kotov,24I.Kravchenko,24N.Kwak,24S.Anderson,25Y.Kubota,25S.J.Lee,25J.J.O’Neill,25S.Patton,25R.Poling,25T.Riehle,25V.Savinov,25and A.Smith251State University of New York at Albany,Albany,New York122222Ohio State University,Columbus,Ohio432103University of Oklahoma,Norman,Oklahoma730194Purdue University,West Lafayette,Indiana479075University of Rochester,Rochester,New York146276Stanford Linear Accelerator Center,Stanford University,Stanford,California94309 7Southern Methodist University,Dallas,Texas752758Syracuse University,Syracuse,New York132449Vanderbilt University,Nashville,Tennessee3723510Virginia Polytechnic Institute and State University,Blacksburg,Virginia24061 11Wayne State University,Detroit,Michigan4820212California Institute of Technology,Pasadena,California9112513University of California,San Diego,La Jolla,California9209314University of California,Santa Barbara,California9310615University of Colorado,Boulder,Colorado80309-039016Cornell University,Ithaca,New York1485317University of Florida,Gainesville,Florida3261118Harvard University,Cambridge,Massachusetts0213819University of Hawaii at Manoa,Honolulu,Hawaii9682220University of Illinois,Champaign-Urbana,Illinois6180121Carleton University,Ottawa,Ontario,Canada K1S5B6and the Institute of Particle Physics,Canada22McGill University,Montr´e al,Qu´e bec,Canada H3A2T8and the Institute of Particle Physics,Canada23Ithaca College,Ithaca,New York1485024University of Kansas,Lawrence,Kansas6604525University of Minnesota,Minneapolis,Minnesota55455Since thefirst observation of the lowest lying charmed baryon,theΛ+c,there have been many measurements made of its exclusive decay channels.As it is difficult to measure the production cross-section of theΛ+c baryons,decay rates are typically presented as branching ratios relative toΛ+c→pK−π+,the most easily observed decay channel.However,fewer than half of theΛ+c hadronic decays are presently accounted for.Measurement of these modes is of practical as well as theoretical interest.Here,we present measurements of the branching fractions ofΛ+c into pK−π+π0,p K0π+π−,and pE2beam−m2Λc is the scaled momentum of theΛ+c candidate.Approximately 60%ofΛ+c baryons from cK0candidates were identified in their decay K0s→π+π−,by reconstructing a secondary vertex from the intersection of two oppositely charged tracks in the r−φplane. The invariant mass of theMode MC Width(MeV)SignalK0191025±40 pK0π027774±52 TABLE I.The number ofΛ+c’s found with x p(Λc)>0.5D∗+→K−π+π+decays that were identified topologically.The reconstruction efficiency of theΛ+c decays has some dependence on the resonant substructure of these states.In the case of the pK−π+mode,the Monte-Carlo generator produced a mixture of non-resonant three-body decay together with∆++K−and pK0→K0s and K0s→π=π−branching fractions.We have considered many possible sources of systematic error in the measurement.The main contributors to the systematic uncertainty came from the following sources:1)Un-certainties in thefitting procedures,which were estimated by looking at the changes in the yields using different orders of polynomial background and different signal widths(15%in the case of pK−π+π0,but much smaller for the other modes),2)uncertainties due to the unknown mix of resonant substructure in the multi-body decays(up to3%depending on the mode),3)uncertainties due toπ0finding(5%),K0sfinding(5%)and trackfinding(1%), and4)uncertainties in the reconstruction efficiency due to the particle identification criteria for protons and kaons(4%).These uncertainties have been added in quadrature to obtain the total systematic uncertainty for each mode,taking into account the fact that many of these tend to cancel in a measurement of ratios of branching fractions.There are three main types of quark decay diagrams that contribute toΛ+c decays.The simplest method is the simple spectator diagram in which the virtual W+fragments inde-pendently of the spectator quark.The second method involves the quark daughters of the W+combining with the remaining quarks.The third method,W-exchange,involves the W+ combining with the initial d quark.Unfortunately all the decay modes under investigation here can proceed by more than one of these decay diagrams,and their decay rates are not amenable to calculation.In conclusion,we have measured new branching fractions of theΛ+c into4decay modes, measured relative to the normalizing modeΛ+c→pK−π+.The results for three of these modes are in agreement with,and more accurate than,previous measurements.We have made thefirst measurement of the decay rate ofΛ+c→pMode Relative Efficiency B/B(pK−π+)Previous MeasurementsK00.2180.46±0.02±0.040.44±0.07±0.05[4]0.55±0.17±0.14[6]0.62±0.15±0.03[7]pK0π00.1150.66±0.05±0.07TABLE II.The measured relative branching fractionsREFERENCES[1]Y.Kubota et al.,Nucl.Inst.and Methods A320,66(1992).[2]R.Brun et al.,CERN/DD/EE/84-11.[3]Review of Particle Properties,R.Barnett et al.,Phys.Rev.D541(1996).[4]P.Avery et al.,Phys.Rev.D43,3499(1991)[5]S.Barlag et al.,Z.Phys.C48,29(1990).[6]J.Anjos et al.,Phys.Rev.D41,801(1990).[7]H.Albrecht et al.,Phys.Lett.B207,109(1988).IVeM5/stnevEIMass (GeV)FIG.1.Invariant mass plots for the5different decay modes of theΛ+c。
OPEN CHARM PRODUCTION CPTH-S492-0197 IN DIFFRACTIVE ep COLLISIONS

1 2
email: diehl@orphee.polytechnique.fr Unit´ e propre 14 du CNRS
1
Introduction
The discovery of diffractive events in ep collisions at HERA [1] has triggered a large amount of experimental and theoretical work and greatly increased our knowledge of the physics of diffraction, or of the pomeron. Several models give a reasonable description of the data at the time being, but we are far yet from a coherent picture of the mechanisms at work in terms of QCD. One can hope that the detailed study of the diffractive final state will lead to further progress in this direction. Charm production looks promising in this respect, as predictions for this process differ widely between various models [2, 3, 4, 5, 6]. In this paper we use the approach due to Landshoff and Nachtmann (LN) to model the soft pomeron by the exchange of two nonperturbative gluons. We present differential cross sections for the diffractive dissociation of a real or virtual photon into a cc ¯-pair. Provided that the invariant mass of the diffractive final state is not too large its cc ¯-component should give a fair approximation of inclusive diffractive charm production. In the following section we give some details of the model and of the calculation, in sec. 3 we present our results, and in sec. 4 we summarise our findings.
Concentrationmeasurement:

Concentration MeasurementBackground:It’s very important to be able to measure the concentration of various substances in a solution. If we use a certain amount of a solution in a reaction, we would really like to know the number of molecules we used, not just the volume we poured in. If we made the solution ourselves, and did it carefully, then we know the concentration, but if we use a solution that someone else made… we have to trust them, or measure it ourselves. If we use a solution that has been sitting around for a long time… the concentration MIGHT still be what is marked on the label, but it might also have changed, if reactions have occurred in the interim.Measuring the concentration of a solution is tricky, since there are no “concentration meters.” We must calculate the concentration from other measurements.We can often measure the concentration of a solution by comparing the properties of the solution to other solutions, where we know the concentrations.•If the solute absorbs visible light, a concentrated solution will have a deeper coloration than a more dilute solution. Two solutions of the same compound that absorb the same amount of light have the same concentration.•Some compounds break into ions (positively and negatively charged bits) in water. A solution of that compound will conduct electricity. The conductivity of the solution will depend upon the number of movable ions. Two solutions of the same compound that conduct equally well have the same concentration.I will give you detailed directions for Part 1 of this lab, but you are going to generate your own procedures for Part 2.Part 1: Finding Concentration using a Colorimeter (Absorbance) Probe: Preparing the solutionsMake five standard NiCl2 solutions. Nickel chloride crystals have water moleculestrapped within them. The mass of water must be considered, or the moles of nickelchloride will be incorrect. The symbol NiCl2•6H2O means that there are six watermolecules for every nickel chloride, so the molecular mass should include those eighteen atoms. You will need to perform the appropriate calculations before you come to class. If you have not done the calculations you will be unable to start the experiment. Check your work with your lab partner.Prepare 50.0 ml of a 0.260M solution of NiCl2• 6H2O.How many grams of the solid do you need to mass out?From that solution (solution 1) prepare 50.0 ml of a 0.200M solution.What volume of solution 1 do you need to use, to make solution 2?You will still have some leftover 0.260M solution for the tests.Put the extra into a test tube labeled #1.From solution 2, prepare 50.0 ml of a 0.160M solution.What volume of solution 2 do you need to use, to make solution 3?You will still have some leftover 0.200M solution for the tests.Put the extra into a test tube labeled #2.From solution 3, prepare 50.0 ml of a 0.120M solution.What volume of solution 3 do you need to use, to make solution 4?You will still have some leftover 0.160M solution for the tests.Put the extra into a test tube labeled #3.From solution 4, prepare 50.0 ml of a 0.100M solution.What volume of solution 4 do you need to use, to make solution 5?You will still have some leftover 0.120M solution for the tests.Put the extra into a test tube labeled #4.Put solution 5 into a test tube labeled #5. (If there is extra, leave it in the flask.) Preparing the Absorbance samples:Fill a cuvette (small container used in the colorimeter device) with each solution. Keep them in order, and put numbered lids on the cuvettes.The cuvettes each contain about 4 ml of liquid.Carefully, blot dry the outside of each cuvette. Try not to scratch the surfaces. Do not touch the smooth sides with your hands.Recording Absorbance data:The colorimeter is used to measure how much light of a given wavelength is absorbed by a sample. If the sample is more concentrated, it absorbs more light. As long as ALL ofthe light is not absorbed, the relationship can be determined. For NiCl2 we will set thecolorimeter to 635nm (red light, well absorbed by the green solution). Before putting any samples in the colorimeter, use the left & right arrows to select 635nm, put a cuvette filled with water into the compartment (see below for directions), and then push CAL to calibrate the device. If you are using one of the older colorimeters I will show you how to calibrate it during lab.To insert a cuvette, hold it by the ridged sides, not the clear sides, and insert it into the square well underneath the cover. The clear sides should be pointing front and back, the ridged sides, left and right. Close the cover.Using the “events with entry” mode on the LabQuest, measure the absorbance of each solution. The “value” to enter is the concentration of the solution. Inspect the data. If there is a definite pattern your solutions were well made. Record your data.Using a sixth cuvette, filled with the solution of unknown concentration, measure its absorbance. Comparing the absorbance measured with the graph you will plot, determine the concentration of the unknown solution.-----------------------------------------------------------------------------------------------------------It’s pretty cool that we can use the colorimeter to find the concentrations of solutions that are colored. But, if a solution is NOT colored, the colorimeter will not be helpful. Fortunately there are other devices that we can use.Part 2: Finding Concentration using a Conductivity Probe:You will need to come up with your own procedures for this part.We would like to find the concentration of salt in seawater. However, since we don’t want to deal with plankton and that sort of thing, we’re going to use a sample of water from a salt-water aquarium instead. As mentioned above, we want to make comparisons to solutions of known concentrations. We have plenty of solid NaCl in our lab. Consider Part 1 of this lab as you plan your Part 2 experiments. What calculations will you need to do?Our conductivity meter will not measure accurately for NaCl solutions with a concentration greater than 0.200M.What do you want to do first?What decisions do you need to make before moving on?How will you know if your data is meaningful?Comparing the conductivity measured with the graph you will plot, determine the concentration of the unknown solution.Recording conductivity data:The conductivity probe is used to measure how conductive the solution is.Select the 0 –20,000 µS setting on the probe box.To measure conductivity, the probe simply needs to be placed in a test tube containing the solution. The opening at the bottom must be fully immersed.Using the “events with entry” mode on the LabQuest, measure the conductivity of all solutions (of known and unknown concentration). Rinse and dry the conductivity probe before each measurement. Record your data.Analysis (and later discussion.)Plot a graph (in your lab notebook) of the absorbance data. It should be at least one-half page in size. What is your independent variable (x-axis)? What is your dependent variable (y-axis)? The scales you use for each variable should be evenly spaced, although your data points will not be. The origin of your graph should be (0,0). Do not offset your graph. Draw a “best-fit” line or curve (as appropriate) to your data.Plot a graph of the conductivity data. Consider the graphing tips above.unknown?What was the concentration of the NiCl2What was the concentration of salt in seawater?Analysis should include a discussion of the sources of systematic error in the experiments.How do these two methods compare? Which method was easier? Which method was more accurate? Which method was more interesting?When do scientists use each of these methods? Do you ever use these methods yourself? (Albeit without the devices) Make lab-to-world connections.Write a clear conclusion paragraph, detailing (but not repeating the entire analysis) what was learned from these experiments.。
Measurement of the FD camera

GAP - 2000 - 010Roma, 1 March 2000Measurement of the FD camera light collection efficiency and uniformityP. Facal San Luis Sezione INFN di Roma II, Roma, Italy and Universidad de Santiago de Compostela, Santiago de Compostela, Spain P. Privitera ` Universita di Roma II, Tor Vergata, and Sezione INFN di Roma II, Roma, ItalyAbstract. Detailed measurements of the light collection efficiency and uniformity of the FD camera with a set up which closely simulate the optics of the FD telescope are presented. We observed that light collection at the pixel edges is very much improved when mercedes are used. The light collection efficiency averaged over the FD focal surface is 93%.1IntroductionThe Fluorescence Detector (FD) camera is composed of a matrix of 20x22 hexagonal pixels, positioned on the focal surface of a spherical mirror. Details of the geometry and the mechanical characteristics of the camera are given in [1]. Hexagonal phototubes are used to instrument the focal surface as fluorescence light detectors. Even if their hexagonal shape represents the best approximation to the pixels geometry, a significant amount of insensitive area is nevertheless present. In fact, some space between PMTs is needed for a safe mechanical packaging on the focal surface; moreover, the effective cathode area is smaller than the area delimited by the PMT glass envelope. A crucial property of the FD camera is its efficiency of collection and uniformity. In fact, large corrections on the energy estimated from the PMT signals would be needed in case of significant losses of light in the pixel borders. 1The systematic uncertainties on the measurement of the cosmic rays energy and direction due to this effect could spoil the performance of the FD. For this reason, the hexagonal phototubes of the AUGER FD camera are complemented by a simplified version of “Winston cones” [2], which maximize light collection and guarantee a sharp transition between adjacent pixels, The mechanical characteristics of these light collectors (mercedes) and their mounting were described in [1]. In this note, we present a measurement of the camera light collection efficiency and uniformity, based on tests of a small prototype of the camera instrumented with 7 phototubes, (the sunflower). The experimental set up used for the measurement is described in Section 2. A light source system, reproducing the fluorescence light and the mirror optics, was moved over the surface of the sunflower. Results of light scans over the surface of the sunflower are presented in Section 3. Conclusions on the camera uniformity are given in Section 4.2The experimental set upA sketch of the experimental set up used for the measurement of the camera uniformity is shown in Fig. 1. A small version of the full size camera body holds seven Philips hexagonal phototubes XP3062, arranged in a sunflower configuration (Fig. 2). High voltage, low voltage and signals from the PMTs are handled by the distribution board [3]. Signals are sampled by an 8-bit flash ADC (CAEN V534) at 20 MHz, and readout by a VME controller Motorola MVME2700. A Xenon flash lamp (Hamamatsu L2360) provides light pulses of approximately 1 µs width with high stability. The time distribution of the light pulse is shown in Fig. 3. The distribution of the light pulse maximum is presented in Fig. 4, showing a stability of 1.5%. The FD detector optics [4] produce a light spot of 1.5 cm diameter on the camera, with light rays angles of incidence in the interval between approximately 10 to 30 degrees. The upper limit is determined by the aperture of the diaphragm while the lower limit results from the shadow of the camera. In order to simulate the optics, the Xenon lamp is attached to a light diffusing cylinder, sketched in Fig. 5. Teflon disks placed inside the cylinder diffuse the light from the Xenon flash lamp. A black disk of 10 cm diameter provides the correct angles of incidence range for light rays passing through the exit hole at the end of the cylinder. The 1.5 cm diameter exit hole simulates the light spot. The 300-400 nm wavelength range corresponding to the fluorescence light is selected by an UG-1 filter placed at the exit hole. The light diffusing cylinder is fixed on a frame which allows X-Y movements over the sunflower surface. The reflecting surface of the light collectors used for the tests presented in this note was obtained glueing aluminized mylar on the mercedes.23Measurement of the camera uniformityThe uniformity of the camera response was measured with the following procedure. The light diffusing cylinder was moved over the sunflower surface in steps of a few mm. The light from the exit hole would hit one or more PMTs depending on its position over the surface. For each step, the signal of each photomultiplier was measured, and normalized to the value obtained when the light spot was positioned on the center of the PMT. Then, the sum of the normalized signals was calculated. For a full light collection efficiency, is expected to be close to unity. A first set of measurements was taken without the light collectors, placing the exit hole of the light diffusing cylinder very close to the PMTs photocathodes. Afterwards, the mercedes were mounted on the small camera body and the light scans were repeated placing the exit hole of the light diffusing cylinder very close to the edges of the light collectors. The results of a scan along a line passing over the mercedes arms are shown in Fig. 6 (see Fig. 2 for the coordinate system). The loss of light in the borders between phototubes is clearly observed. Another scan which passes over the mercedes vertices, where the light loss is maximal, is presented in Fig. 7. On the basis of the results obtained, we can draw the following conclusions: • significant losses of light, up to 70%, are present in the pixel borders when the light collectors are not used. Also, the response within the same pixel shows a typical structure, which can be attributed to the shape of the collecting electric field at the edges of the hexagonal photocathodes. • the light collectors are efficiently recuperating the light loss, which is reduced to less than 15% even in the worst case with the spot over a mercedes vertex. The uniformity within the same pixel is also improved, since light rays which were hitting the photocathode borders are now redirected by reflection over the mercedes in the central region of the photocathode. A complete map of the sunflower equipped with light collectors was performed. In Fig. 8 we present the measured as a function of the light spot position over the sunflower. In the region covered by PMTs, a value always larger than 85% is measured, with ’bumps’ corresponding to the PMTs positions on the surface. Several scans are shown in Fig. 9-12. To guide the eye, lines connecting the measured efficiency as a function of the step movement are presented. The contribution from individual PMTs is also shown. In Fig. 13 we present the distribution of the efficiency for the subset of measurements which were performed on the area covered by the central pixel of the sunflower. Notice that the measurements were performed with constant density over the surface of the sunflower. Thus, the average efficiency of 93% obtained from this distribution corresponds to the light collection efficiency averaged over the FD focal surface.4ConclusionsWe have performed detailed measurements of the response to light of the FD 3camera with a set up which closely simulate the optics of the FD telescope. We observed that light collection at the edges of the pixel is very much improved when mercedes are used. Also, the uniformity of response to light improved since mercedes cover the photocathode edges, where photoelectron collection is less uniform. The light collection efficiency changes from 100%, when the spot is over the pixel center, to a minimum value of 85% in the worst case with the spot over a mercedes vertex. The light collection efficiency averaged over the FD focal surface is found to be 93%. In terms of shower energy reconstruction, an average correction of 7% to the signal measured by each pixel would already reduce the systematic uncertainty on the energy measurement to the 5% level. It is conceivable that more elaborated corrections depending on the shower image position over the focal surface will further reduce the systematic uncertainty. We acknowledge the suggestion given by Paul Sommers of using a light diffusing cylinder to simulate the FD optics. The technical contributions of Francesco Bracci, Gianni Vitali and Enrico Tusi were essential for the realization of this work.References[1] C. Aramo et al., The camera of the AUGER Fluorescence Detector, Auger Tech. Note GAP-99-027 [2] R. Winston and W.T.Welford, High collection nonimaging optics, Academic Press, 1989 [3] R. Cardarelli et al., The baseline design of the backplane distribution system of the FD camera, Auger Tech. Note GAP-99-029 [4] G. Matthiae and P. Privitera, The Schmidt telescope with corrector plate, Auger Tech. Note GAP-98-0394Figure 1: Sketch of the experimental apparatus used for the measurement of the camera uniformity.5Figure 2: The sunflower geometry. The grey circle on the upper right corner represents the lightspot.6Figure 3: Time distribution of the Xenon flash lamp light pulse. The histogram shows the average over 1000 pulses. The dotted line indicates the pedestal level.Figure 4: Distribution of the light pulse maximum.7Figure 5: The light diffusing cylinder used to simulate the FD optics.8εFigure 6: Measurement of the light collection efficiency along a line passing over the mercedes arms. The full dots represents the measurements performed with mercedes, while the open dots the measurements without mercedes .9εFigure 7: Measurement of the light collection efficiency along a line passing over the mercedes verteces. The full dots represents the measurements performed with mercedes, while the open dots the measurements without mercedes .10Figure8:Light collection efficiency as a function of the x and y position of the light spot overthe sunflower.Seven bumps corresponding to the PMTs are visible.ε217ε2175Figure9:Measurements of the light collection efficiency along lines parallel to the y axis(full lines).The dashed lines represent the contribution from individual pixels.25736ε23576Figure 10:Measurements of the light collection efficiency along lines parallel to the y axis (fulllines).The dashed lines represent the contribution from individual pixels.23ε23154Figure 11:Measurements of the light collection efficiency along lines parallel to the x axis (fulllines).The dashed lines represent the contribution from individual pixels.154ε76154Figure12:Measurements of the light collection efficiency along lines parallel to the x axis(full lines).The dashed lines represent the contribution from individual pixels.Figure13:Distribution of the light collection efficiency in the central pixel.。
半径约束最小二乘圆拟合方法及其误差分析_刘珂

第17卷第5期2006年5月光电子#激光Journal of O p toelectronics#LaserV o l.17N o.5M ay2006半径约束最小二乘圆拟合方法及其误差分析*y刘珂**,周富强,张广军(北京航空航天大学仪器科学与光电工程学院,北京100083)摘要:针对基于线结构光视觉检测类圆工件三维测量中的光条圆弧特征数据所占整圆比例偏小,提出了基于半径约束最小二乘圆拟合方法。
详细地分析了样本特征数据噪声对圆心定位精度的影响,并进行了仿真实验。
实验结果表明,在光条圆弧特征数据所占整圆比例偏小的条件下,半径约束最小二乘圆拟合方法可以有效地提高圆中心定位精度。
关键词:最小二乘法;半径约束;圆拟合;线结构光;误差分析中图分类号:T P391文献标识码:A文章编号:1005-0086(2006)05-0604-04Radius C onstraint Leas-t square Circle Fitting Method and Error AnalysisLIU Ke**,ZH OU Fu-qiang,ZH ANG Guang-jun(School of Instrument Science&O pt oelectr onics Eng ineer ing,Beijing U niv ersity of A ero nautics and A s-t ronautics,Beijing100083,China)Abstract:In the process of3-D measurement of the workpiece in shape of arc with struc-tu red light vision sensor,feature data of the stru ctu red light arc is inadequ ate compared with the whole circle,which results in the location of the circle centre bein g of low prec-i sion.Leas-t squares method based on radius constraint is proposed in the paper to solve the problem.Influence of the noise in sample data the on the locating of circle centre is ana-lyzed in detail,and simulated experimen ts are implem ented.Results of experiments show that under the circumstan ces that featu re data of the structured light arc is inadequate com-pared with the whole circle,the method proposed above can improve the accuracy of circle fitting efficiently.Key words:leas-t squares m ethod;radius constrai ned;li ne structured li ght;anal ysis of error1引言各种零部件定位圆孔几何中心的定位精度对零部件的成功安装以及物体的整体定位,有着重要的意义。
3B Scientific 莱茵悬杆实验设备说明书

3B S c i e n t i f i c ® E x p e r i m e n t s ...g o i n g o n e s t e p f u r t h e r1m 2m 1m 1m 2jrdSUMMARYThe central component of a Cavendish torsion balance is a sensitive torsional pendulum with a pair of small lead spheres attached to it. Two larger lead spheres are then placed near these two small balls in order to attract them. The position of the large spheres thus determines the equilibrium position of the torsional pendulum. If the two large spheres are then moved to a second position which issymmetrical with the first with respect to the two small balls, the torsional pendulum will adopt a new equilibrium position after a short period of settling. By measuring the geometry of the set-up in both E X PERIMEN T PROCEDURE• D etermine the initial equilibrium positi -on of the torsional pendulum.• R ecord the oscillation of the torsional pendulum about the final equilibrium position and determine the period.• D etermine where the final equilibrium position is.Gravitational constant MECh A NIC S / MEA SURINg PROCEDURES OBJECTIVEMeasure the gravitational force and determine the gravitational constant using Cavendish torsion balanceBASIC PRINCIPL When measuring the gravitational force between two masses in a laboratory, it is inevitably thecase that all other masses in the vicinity have a disturbing effect on the results. The Cavendish balance largely gets around this problem since two measurements are made with the masses sym metrically positioned.an hour. A mirror attached to the torsional pendulum can be used to set up a light pointer so that the oscillations are easy to follow with the naked eye. This makes the necessary adjustment and calibration of the balance much easier.UE1010300UE1010300Quantity DescriptionNumber 1Cavendish Torsion Balance 10033371Laser Diode, Red 10032011Barrel Foot, 1000 g 10028341Universal Clamp10028301Stainless Steel Rod 100 mm1002932Additionally recommended:1Callipers, 150 mm 10026011Electronic Scale 5000 g1003434The central component of a Cavendish torsion balance is a sensitive torsi-onal pendulum with a pair of small lead spheres attached to it. Two largerlead spheres are then placed near these two small balls in order to attract them. The position of the large spheres thus determines the equilibrium position of the torsional pendulum. If the two large spheres are then moved to a second position which is symmetrical with the first with respect to the two small balls, the torsional pendulum will adopt a new equilibrium posi-tion after a short period of settling. By measuring the geometry of the set-up in both positions, it is possible to determine the gravitational constant. The decisive factor in this is the equilibrium between the gravitational force and the restoring torque of the torsional pendulum.The gravitational force is given by the following:(1)G : Gravitational constant,m 1: Mass of one small lead sphere, m 2: Mass of one large lead sphere,d : Distance between small and large lead spheres at the position where themeasurement is madeThe force deflects the torsional pendulum from its equilibrium position when the two large spheres are in position for the measurement. The deflecting torque is (2) r : Distance of small lead sphere from its mounting point on thesupporting beamIf the torsional pendulum is deflected by an angle ϕ, there is a restoring torque(3)D : Torsion coefficient of tungsten wireThis acts due to the tungsten wire from which the support beam of the torsional balance is suspended. In the equilibrium position, M 1 and M 2 are equal.The torsional coefficient D can be determined from the period of oscillation T for the oscillation of the torsional pendulum about its equilibrium posi-tion.(4) The moment of inertia J comprises the moment of inertia J 1 of the two small spheres and the moment of inertia J K of the supporting beam (5)m B : Mass of support beama ,b : Length and width of support beam.For the two large lead spheres, there should be two symmetrical positions where measurements are made. The angles of deflection in these two posi-tions are ϕ and ϕ’ and the two corresponding deflecting torques are equal but in opposite directions. In equilibrium, equations (2) and (3) therefore imply the following:(6)In the course of the experiment the oscillations of the torsional pendulum are measured using a capacitive differential sensor, which suppresses noise and vibrational components of the signal to a large extent. The tungsten wire from which the pendulum is made is chosen to be so thin that the period of oscillation is of the order of a few minutes, meaning that several oscillations about the equilibrium position may be observed in the space ofFig. 2: Angle of deflection of torsional pendulum as a function of time when the measurement position of the two large lead spheres has been changed twiceFig. 1: Schematic of measurement set-up for the Cavendish torsional balanceF =G ⋅m 1⋅m 2d 2M 1=2⋅F ⋅rM 2=D ⋅ϕD =J ⋅4π2T 2J =2⋅m 1⋅r 2+m B12⋅a 2+b 2()4⋅F ⋅r =D ⋅ϕ−ϕ'()=D ⋅ΔϕG =Δϕm 2⋅d 2⋅π2T 2⋅2⋅r +112⋅m B m 1⋅a 2+b 2r ⎛⎝⎜⎞⎠⎟E VA LUAT IONBy rearranging equations (1), (4), (5) and (6):.This does not take into account that the two small spheres are alsoattracted by the more distant large sphere, so that the torque on the torsional pendulum is somewhat reduced in comparison with the calcu-lations made so far. It is not difficult to introduce correction for this into equation (2), since all the distance are known.。
测量

Measurement conditions
• Always take measurement before fitting on model/dummy. • Always take both left and right side measurements, e.g. Length from shoulder, Sleeve opening, etc. • Always take stretched measurements (ex: Minimum neck stretched) at last, after taking all the other measurements.
Measurement conditions
• Always lay the opening (ex: sleeve opening, leg opening , bottom, etc) at a position with the side seams not at the side, but slightly shifted.
Tops
Measurement tips
• Neckline
For hemmed neck with extending the same fabric from the main body, i.e. no binding/ribs, the basic line will be along the neck edge.
First measurement of the $pi^+pi^-$ atom lifetime

a r X i v :h e p -e x /0504044v 2 20 M a y 2005First measurement of the π+π−atom lifetimeB.Adeva p ,L.Afanasyev ℓ,∗,M.Benayoun e ,A.Benelli q ,Z.Berka b ,V .Brekhovskikh o ,G.Caragheorgheopol m ,T.Cechak b ,M.Chiba k ,S.Constantinescu m ,C.Detraz a ,D.Dreossi g ,D.Drijard a ,A.Dudarev ℓ,I.Evangelou d ,M.Ferro-Luzzi a ,M.V .Gallas p ,a ,J.Gerndt b ,R.Giacomich g ,P.Gianotti f ,D.Goldin q ,F.G´o mez p ,A.Gorin o ,O.Gorchakov ℓ,C.Guaraldo f ,M.Hansroul a ,R.Hosek b ,M.Iliescu f ,m ,V .Karpukhin ℓ,J.Kluson b ,M.Kobayashi h ,P.Kokkas d ,V .Komarov ℓ,V .Kruglov ℓ,L.Kruglova ℓ,A.Kulikov ℓ,A.Kuptsov ℓ,I.Kurochkin o ,K.-I.Kuroda ℓ,mberto g ,naro a ,f ,V .Lapshin o ,R.Lednicky c ,P.Leruste e ,P.Levi Sandri f ,A.Lopez Aguera p ,V .Lucherini f ,T.Maki j ,N.Manthos d ,I.Manuilov o ,L.Montanet a ,J.-L.Narjoux e ,L.Nemenov a ,ℓ,M.Nikitin ℓ,T.N´u ˜nez Pardo p ,K.Okada i ,V .Olchevskii ℓ,A.Pazos p ,M.Pentia m ,A.Penzo g ,J.-M.Perreau a ,C.Petrascu f ,m ,M.Pl´o p ,T.Ponta m ,D.Pop m ,G.F.Rappazzo g ,A.Rodriguez Fernandez p ,A.Romero p ,A.Ryazantsev o ,V .Rykalin o ,C.Santamarina p ,q ,a ,J.Saborido p ,J.Schacher r ,Ch.P.Schuetz q ,A.Sidorov o ,J.Smolik c ,F.Takeutchi i ,A.Tarasov ℓ,L.Tauscher q ,M.J.Tobar p ,S.Trusov n ,V .Utkin ℓ,O.V´a zquez Doce p ,P.V´a zquez p ,S.Vlachos q ,V .Yazkov n ,Y .Yoshimura h ,M.Zhabitsky ℓ,P.Zrelov ℓa CERN,Geneva,Switzerland b Czech Technical University,Prague,Czech Republicc Institute of Physics ACSR,Prague,Czech Republicd IoanninaUniversity,Ioannina,Greece e LPNHEdes Universites Paris VI/VII,IN2P3-CNRS,France f INFN -Laboratori Nazionali di Frascati,Frascati,Italyg INFN -Trieste and Trieste University,Trieste,Italyh KEK,Tsukuba,Japan i Kyoto Sangyo University,Kyoto,JapanPreprint submitted to Physics Letters B 7February 2008j UOEH-Kyushu,Japank Tokyo Metropolitan University,JapanℓJINR Dubna,Russiam IFIN-HH,National Institute for Physics and Nuclear Engineering,Bucharest,Romania n Skobeltsin Institute for Nuclear Physics of Moscow State University Moscow,Russiao IHEP Protvino,Russiap Santiago de Compostela University,Spainq Basel University,Switzerlandr Bern University,Switzerland1IntroductionThe aim of the DIRAC experiment at CERN[1]is to measure the lifetime of pi-onium,an atom consisting of aπ+and aπ−meson(A2π).The lifetime is dom-inated by the charge-exchange scattering process(π+π−→π0π0)1and is thus related to the relevant scattering lengths[4].The partial decay width of the atomic ground state(principal quantum number n=1,orbital quantum number l=0)is [2,5,6,7,8,9]Γ1S=19α3p|a0−a2|2(1+δ)(1)withτ1S the lifetime of the atomic ground state,αthefine-structure constant,p the π0momentum in the atomic rest frame,and a0and a2the S-waveππscattering lengths for isospin0and2,respectively.The termδaccounts for QED and QCD corrections[6,7,8,9].It is a known quantity(δ=(5.8±1.2)×10−2)ensuring a1%accuracy for Eq.(1)[8].A measurement of the lifetime therefore allows to obtain in a model-independent way the value of|a0−a2|.Theππscattering lengths a0,a2have been calculated within the framework of Standard Chiral Perturbation Theory[10]with a precision better than2.5%[11](a0=0.220±0.005,a2=−0.0444±0.0010,a0−a2=0.265±0.004in units of inverse pion mass)and lead to the predictionτ1S=(2.9±0.1)×10−15s.The Generalized Chiral Perturbation Theory though allows for larger a-values[12].Model independent measurements of a0have been done using K e4decays[13,14].Oppositely charged pions emerging from a high energy proton-nucleus colli-sion may be either produced directly or stem from strong decays(”short-lived”sources)and electromagnetic or weak decays(”long-lived”sources)of interme-diate hadrons.Pion pairs from“short-lived”sources undergo Coulombfinal state interaction and may form atoms.The region of production being small as compared to the Bohr radius of the atom and neglecting strongfinal state interaction,the cross sectionσn A for production of atoms with principal quantum number n is related to the inclusive production cross section for pion pairs from”short lived”sources without Coulomb correlation(σ0s)[15]:dσn AM A |ΨC n( r∗=0)|2d2σ0sd p+d p−=|ΨC− k∗( r∗)|2d2σ0s2For the sake of clarity we use the symbol Q for the experimentally reconstructed and q for the physical relative momentum.3the Coulomb correlation and at r∗=0coincides with the Gamov-Sommerfeld factor A C(q)with q=| q|[17]:A C(q)=2πmπα/qN CC =σtot Aπ∞n=11q0A C(q)d3q=k th(q0).(5)Eq.(5)defines the theoretical k-factor.Throughout the paper we will useq0=2MeV/c and k th(q0)=0.615.(6) In order to account for thefinite size of the pion production region and of the two-pionfinal state strong interaction,the squares of the Coulomb wave functions in Eqs.(2)and(3)must be substituted by the square of the complete wave functions, averaged over the distance r∗and the additional contributions fromπ0π0→A2πas well asπ0π0→π+π−[17].It should be noticed that these corrections essentially cancel in the k-factor(Eq.(5))and lead to a correction of only a fraction of a percent.Thusfinite size corrections can safely be neglected for k th.Once produced,the A2πatoms propagate with relativistic velocity(average Lorentz factor¯γ≈17in our case)and,before they decay,interact with target atoms, whereby they become excited/de-excited or break up.Theπ+π−pairs from break-up(atomic pairs)exhibit specific kinematical features which allow to identify them experimentally[15],namely very low relative momentum q and q L(the component of q parallel to the total momentum p++ p−)as shown in Fig.1.After break-up,the atomic pair traverses the target and to some extent loses these features by multiple scattering,essentially in the transverse direction,while q L is almost not affected. This is one reason for considering distributions in Q L as well as in Q when analyz-ing the data.Excitation/de-excitation and break-up of the atom are competing with its decay. Solving the transport equations with the cross sections for excitation and break-up, [20,21,22,23,24,25,26,27,28,29,30,31]leads to a target-specific relation between break-up probability and lifetime which is estimated to be accurate at the1%level [22,32,33].Measuring the break-up probability thus allows to determine the life-time of pionium[15].410100103104105q , |q L | [MeV/c ]Fig.1.Relative momentum distributions (q ,q L )for atomic π+π−pairs at the point of break-up and at the exit of the target.Note that q L is almost not affected by multiple scat-tering in the target.The first observation of the A 2πatom [34]has allowed to set a lower limit on its lifetime [18,19]of τ>1.8×10−15s (90%CL).In this paper we present a deter-mination of the lifetime of the A 2πatom,based on a large sample of data taken in 2001with Ni targets.2The DIRAC experimentThe DIRAC experiment uses a magnetic double-arm spectrometer at the CERN 24GeV/c extracted proton beam T8.Details on the set-up may be found in [35].Since its start-up,DIRAC has accumulated about 15’000atomic pairs.The data used for this work were taken with two Ni foils,one of 94µm thickness (76%of the π+π−data),and one of 98µm thickness (24%of the data).An extensive description of the DIRAC set-up,data selection,tracking,Monte Carlo procedures,signal extraction and a first high statistics demonstration of the feasibility of the lifetime measurement,based on the Ni data of 2001,have been published in [36].The set-up and the definitions of detector acronyms are shown in Fig.2.The main selection criteria and performance parameters [36]are recalled in the following.Pairs of oppositely charged pions are selected by means of Cherenkov,preshower and muon counters.Through the measurement of the time difference between the vertical hodoscope signals of the two arms,time correlated (prompt)events (σ∆t =185ps)can be distinguished from accidental events (see [36]).The resolution of the three components of the relative momentum Q of two tracks,transverse and parallel5o pFig.2.Schematic top view of the DIRAC spectrometer.Upstream of the magnet:target,mi-crostrip gas chambers(MSGC),scintillatingfiber detectors(SFD),ionization hodoscopes (IH)and iron shielding.Downstream of the magnet:drift chambers(DC),vertical and hor-izontal scintillation hodoscopes(VH,HH),gas Cherenkov counters(Ch),preshower detec-tors(PSh)and,behind the iron absorber,muon detectors(Mu).to the c.m.flight direction,Q x,Q y and Q L,is about0.5MeV/c for Q≤4MeV/c. Due to charge combinatorials and inefficiencies of the SFD,the distributions for the transverse components have substantial tails,which the longitudinal component does not exhibit[37].This is yet another reason for analyzing both Q and Q L distributions.Data were analyzed with the help of the DIRAC analysis software package ARI-ANE[39].The tracking procedures require the two tracks either to have a common vertex in the target plane(“V-tracking”)or to originate from the intersect of the beam with the target(“T-tracking”).In the following we limit ourselves to quoting results obtained with T-tracking.Results obtained with V-tracking do not show significant differences,as will be shown later.The following cuts and conditions are applied(see[36]):•at least one track candidate per arm with a confidence level better than1%and a distance to the beam spot in the target smaller than1.5cm in x and y;•“prompt”events are defined by the time difference of the vertical hodoscopes in the two arms of the spectrometer of|∆t|≤0.5ns;•“accidental”events are defined by time intervals−15ns≤∆t≤−5ns and 7ns≤∆t≤17ns,determined by the read-out features of the SFD detector (time dependent merging of adjacent hits)and exclusion of correlatedπ−p pairs.[36];•protons in“prompt”events are rejected by time-of-flight in the vertical ho-doscopes for momenta of the positive particle below4GeV/c.Positive particles with higher momenta are rejected;•e±andµ±are rejected by appropriate cuts on the Cherenkov,the preshower and6the muon counter information;•cuts in the transverse and longitudinal components of Q are Q T≤4MeV/c and |Q L|<15MeV/c.The Q T cut preserves98%of the atomic signal.The Q L cut preserves data outside the signal region for defining the background;•only events with at most two preselected hits per SFD plane are accepted.This provides the cleanest possible event pattern.3AnalysisThe spectrometer including the target is fully simulated by GEANT-DIRAC[38], a GEANT3-based simulation code.The detectors,including read-out,inefficiency, noise and digitalization are simulated and implemented in the DIRAC analysis code ARIANE[39].The triggers are fully simulated as well.The simulated data sets for different event types can therefore be reconstructed with exactly the same procedures and cuts as used for experimental data.The different event types are generated according to the underlying physics. Atomic pairs:Atoms are generated according to Eq.(2)using measured total mo-mentum distributions for short-lived pairs.The atomicπ+π−pairs are generated according to the probabilities and kinematics described by the evolution of the atom while propagating through the target and by the break-up process(see[40]). Theseπ+π−pairs,starting from their spatial production point,are then propagated through the remaining part of the target and the full spectrometer using GEANT-DIRAC.Reconstruction of the track pairs using the fully simulated detectors and triggers leads to the atomic pair distribution dn MCA/dQ.Coulomb correlatedπ+π−pairs(CC-background):The events are generated ac-cording to Eqs.(3,4)using measured total momentum distributions for short-lived pairs.The generated q-distributions are assumed to follow phase space modified bythe Coulomb correlation function(Eq.(4)),dN genCC /dq∝q2×A C(q).Processingthem with GEANT-DIRAC and then analyzing them using the full detector and trigger simulation leads to the Coulomb correlated distribution dN MCCC/dQ.Non-correlatedπ+π−pairs(NC-background):π+π−pairs,where at least one pion originates from the decay of a”long-lived”source(e.g.electromagnetically or weakly decaying mesons or baryons)do not undergo anyfinal state interactions.Thus they are generated according to dN genNC /dq∝q2,using slightly softer momen-tum distributions than for short-lived sources(difference obtained from FRITIOF-6).The Monte Carlo distribution dN MCNC/dQ is obtained as above.Accidentalπ+π−pairs(acc-background):π+π−pairs,where the two pions orig-7inate from two different proton-nucleus interactions,are generated according to dN gen acc /dq ∝q 2,using measured momentum distributions.The Monte Carlo distri-bution dN MC acc /dQ is obtained as above.All the Monte Carlo distributions are normalized, Q max 0(dN MC i /dQ )dQ =N MC i ,i =CC,NC,acc ,with statistics about 5to 10times higher than theexperimental data;similarly for atomic pairs (n MC A ).The measured prompt distributions are approximated by appropriate shape func-tions.The functions for atomic pairs,F A (Q ),and for the backgrounds,F B (Q ),(analogously for Q L )are defined as:F A (Q )=n rec A dQF B (Q )=N rec CC dQ +N rec NCdQ +ωacc N prdQ (7)with n rec A ,N rec CC ,N rec NC the reconstructed number of atomic pairs,Coulomb-andnon-correlated background,respectively,and ωacc the fraction of accidental back-ground out of all prompt events N pr .Analyzing the time distribution measured with the vertical hodoscopes (see [36])we find ωacc =7.1%(7.7%)for the 94µm (98µm)data sets [36,37]and keep it fixed when fitting.The χ2function for Q (analogously for Q L )to minimize isχ2=νmax νmin dN pr dN prFig.3.Top:Experimental Q and Q L distributions after subtraction of the prompt accidental background,andfitted Monte Carlo backgrounds(dotted lines).The peak at Q=4MeV/c is due to the cut Q T≤4MeV/c.Bottom:Residuals after background subtraction.The dotted lines represent the expected atomic signal shape.The bin-width is0.25MeV/c. Table1Fit results(94and98µm targets together,background shapes from Monte Carlo(MC))forthe parameters N recCC (total number of CC-events),N recNC(total number of NC events)andn rec A(atomic pairs)and deduced results for the number of atomic pairs from the residu-als(n residualA )and the number of CC-background events in the signal region(N sigCC).MC-a:backgroundfit excluding the signal region.MC-b:fit of the entire momentum range includ-ing Monte Carlo shape for atomic pairs(“shapefit”).The cuts were at Q cut=4MeV/c and Q L,cut=2MeV/c.Q and Q L-distributions werefitted together.The normalizedχ2 were0.9for MC-a and MC-b.N recCC N recNCn residualAn rec A N sig CCQQ LMC-b374282±3561562136530±294106549±1014 same same same82345±783number of atomic pairs,n residualA and of Coulomb correlated background events,N sig CC.Results offits for Q and Q L together are shown in Table1.CC-background and NC-or acc-backgrounds are distinguishable due to their dif-ferent shapes,most pronounced in the Q L distributions(see Fig.3,top).Acciden-tal and NC-background shapes are almost identical for Q and fully identical for Q L(uniform distributions).Thus,the errors in determining the accidental back-groundωacc are absorbed infitting the NC background.The correlation coefficient between CC and NC background is−99%.This strong correlation leads to equalerrors for N recCC and N recNC.The CC-background is determined with a precision better9than1%.Note that the difference between all prompt events and the background is N pr−N rec CC−N rec NC−ωacc N pr=6590,hence very close to the number of residualatomic pairs(n residualA )as expected.This relation is also used as a strict constraintforfits outside of the signal region(>),N>pr−N rec>CC −N rec>NC−(ωacc N pr)>=0and,hence,thefit requires only one free parameter,N rec>CC.Second,the atomic pair signal may be directly obtained by minimizing Eq.(8)over the full range and including the Monte Carlo shape distribution F A(“shapefit”). The signal strength has to be the same for Q and Q L.The result for the signal strength n rec A as well as the CC-background below the cuts,N sig CC,are shown in Table1.The errors are determined by MINOS[41].The consistency between the analysis in Q with the one in Q L establishes the cor-rectness of the Q T reconstruction.A2Dfit in the variables(Q L,Q T)confirms the results of Table1.4Break-up probabilityIn order to deduce the break-up probability,P br=n A/N A,the total number of atomic pairs n A and the total number of produced A2πatoms,N A,have to be known.None of the two numbers is directly measured.The procedure of obtaining the two quantities requires reconstruction efficiencies and is as follows.Number of atomic pairs:Using the generator for atomic pairs a large number ofevents,n genA ,is generated in a predefined large spatial acceptance windowΩgen,propagated through GEANT-DIRAC including the target and reconstructed along the standard procedures.The total number of reconstructed Monte Carlo atomicpairs below an arbitrary cut in Q,n MC−recA (Q≤Q cut)defines the reconstructionefficiency for atomic pairsǫcut A=n MC−recA (Q≤Q cut)/n genA.The total number ofatomic pairs is obtained from the measured pairs by n A=n rec A(Q≤Q cut)/ǫcut A. Number of produced A2πatoms:Here we use the known relation between pro-duced atoms and Coulomb correlatedπ+π−pairs(CC-background)of Eq.(5).Us-ing the generator for CC pairs,N genCC events,of which N genCC(q≤q0)(see Eq.6)have q below q0,are generated into the same acceptance windowΩgen as for atomic pairs and processed analogously to the paragraph above to provide the number of reconstructed CC-events below the same arbitrary cut in Q as for atomic pairs,N MC−rec CC (Q≤Q cut).These CC-events are related to the originally generated CC-events below q0throughǫcut CC=N MC−recCC (Q≤Q cut)/N genCC(q≤q0).The numberof produced atoms thus is N A=k th(q0)N rec CC(Q≤Q cut)/ǫcut CC(see Eq.(6)).10k(Q cut)factors as a function of cuts in Q and Q L for the94and98µm thick Ni targets,and the weighted average of the two for a relative abundance of76%(94µm)and24%(98µm).Q cut=2MeV/cQ cut=3MeV/cQ cut=4MeV/cQ L,cut=1MeV/cQ L,cut=2MeV/cN A =n rec A(Q≤Q cut)ǫcut CC.(9)In Table2the k-factors are listed for different cuts in Q and Q L for the two target thicknesses(94µm and98µm)and the weighted average of the two,corresponding to their relative abundances in the Ni data of2001.The accuracy is of the order of one part per thousand and is due to Monte Carlo statistics.With the k-factors of Table2and the measurements listed in Table1,the break-up probabilities of Table3are obtained.The simultaneousfit of Q and Q L with the atomic shape results in a single value.The break-up probabilities from Q and Q L agree within a fraction of a percent.The values from shapefit and from backgroundfit are in perfect agreement(see Table 1).We adopt the atomic shapefit value of P br=0.447±0.023stat,because thefit covers the full Q,Q L range and includes correlations between n rec A and N sig CC. Analyzing the data with three allowed hit candidates in the SFD search window in-stead of two,results in more atomic pairs(see Ref.[36],T-tracking).The break-up probabilities obtained are0.440±0.024and0.430±0.021for Q and Q L,respec-tively.They are not in disagreement with the adopted value of0.447.Despite the larger statistics,the accuracy is not improved,due to additional background.This background originates from additional real hits in the upstream detectors or from electronic noise and cross-talk.This has been simulated and leads essentially to a reduced reconstruction efficiency but not to a deterioration of the reconstruction quality.The additional sources of systematic uncertainties lead us not to consider this strategy of analysis further on.V-tracking provides a slightly different data sample,different k-factors and differ-ent signal strengths and CC-background.The break-up probability,however,does not change significantly and is P V−trackingbr=0.453±0.025stat,only0.3σoff from11Break-up probabilities for the combined Ni2001data,based on the results of Table1and the k-factors of Table2for the cuts Q cut=4MeV/c and Q L,cut=2MeV/c.Errors are statistical.Q6509±33082289±8730.445±0.0236530±294106549±10040.447±0.023the adopted value0.447.The break-up probability has to be corrected for the impurities of the targets.Thus, the94µm thick target has a purity of only98.4%,while the98µm thick target is 99.98%pure.The impurities(C,Mg,Si,S,Fe,Cu)being mostly of smaller atomic number than Ni lead(for the weighted average of both targets)to a reduction of the break-up probability of1.1%as compared to pure Ni,assuming a lifetime of3fs. Therefore,the measured break-up probability has to be increased by0.005in order to correspond to pure Ni.Thefinal result is:P br=0.452±0.023stat.(10) 5Systematic errorsSystematic errors may occur through the analysis procedures and through physical processes which are not perfectly under control.We investigatefirst procedure-induced errors.The break-up probability will change,if the ratio N recCC /N rec NC depends on thefitrange.If so,the Monte Carlo distributions do not properly reproduce the mea-sured distributions and the amount of CC-background may not be constant.In Fig.4the dependence is shown for thefits in Q,Q L and both together.The ra-tio is reasonably constant within errors,with the smallest errors for afit range of Q=Q L=15MeV/c.At this point the difference between Q and Q Lfits leads to a difference in break-up probability of∆P CCbr=0.023.Consistency of the procedure requires that the break-up probability does not depend on Q cut.In Figure5the dependence on the cut is shown for break-up probabilitiesdeduced from n residualA .There is a systematic effect which,however,levels off forlarge cut momenta.This dependence indicates that the shape of the atomic pair signal as obtained from Monte Carlo(and used for the k-factor determination)is not in perfect agreement with the residual shape.This may be due to systematics12in the atomic pair shape directly and/or in reconstructed CC-background for small relative momenta.The more the signal is contained in the cut,the more the P br values stabilize.As a consequence,we chose a cut that contains the full signal (see Eq.(10)).This argument is also true for sharper cuts in Q T than the one from the event selection.Cut momenta beyond the maximum cut of Figure 5would only test background,as the signal would not change anymore.To investigate whether the atomic pair signal shape is the cause of the above cut dependence,we studied two extreme models for atom break-up:break-up only from the 1S -state and break-up only from highly excited states.The two extremes result in a difference in break-up probability of ∆P shape br =0.008.6789Q, |Q L | [MeV/c ]N C /N n C Fig. 4.Ratio of CC-background overNC-background as a function of fitrange.Fig.5.P br as a function of cut momen-tum for Q and Q L .Sources of systematic errors may also arise from uncertainties in the genuine phys-ical process.We have investigated possible uncertainties in multiple scattering as simulated by GEANT by changing the scattering angle in the GEANT simulation by ±5%.As a result,the break-up probability changes by 0.002per one percent change of multiple scattering angle.In fact we have measured the multiple scatter-ing for all scatterers (upstream detectors,vacuum windows,target)and found nar-rower angular distributions than expected from the standard GEANT model [42].This,however,may be due also to errors in determining the thickness and material composition of the upstream detectors.Based on these studies we conservatively attribute a maximum error of +5%and -10%to multiple scattering.Another source of uncertainty may be due to the presence of unrecognized K +K −and ¯p p pairs that would fulfill all selection criteria [43].Such pairs may be as abun-dant as 0.5%and 0.15%,respectively,of π+π−pairs as estimated for K +K −with FRITIOF-63and for ¯p p from time-of-flight measurements in a narrow momentumTable4Summary of systematic effects on the measurement of the break-up probability P br.Ex-treme values have been transformed intoσassuming uniform distributions.sourceσ+0.012/-0.012signal shape±0.002+0.01/-0.02K+K−and¯p p+0−0.023+0/−0.03.6Lifetime of PioniumThe lifetime may be deduced on the basis of the relation between break-up prob-ability and lifetime for a pure Ni target(Fig.6).This relation,estimated to be ac-curate at the1%level,may itself have uncertainties due to the experimental con-ditions.Thus the target thickness is estimated to be correct to better than±1µm, which leads to an error in the lifetime(for P br=0.45)smaller than±0.01fs,less than1%of the expected lifetime and thus negligible.The result for the lifetime is τ1S= 2.91+0.45−0.38}stat+0.19−0.49}syst ×10−15s= 2.91+0.49−0.62 ×10−15s.(12) The errors are not symmetric because the P br−τrelation is not linear,and because finite size corrections and heavy particle admixtures lead to possible smaller values of P br.The accuracy achieved for the lifetime is about+17%,almost entirely due to statistics and−21%,due to statistics and systematics in roughly equal parts. With full statistics(2.3times more than analysed here)the statistical errors may be reduced accordingly.The two main systematic errors(particle admixtures andfinite size correction)will be studied in more detail in the future program of DIRAC.m−1π. Using Eq.(1),the above lifetime corresponds to|a0−a2|=0.264+0.033−0.020Fig.6.Break-up probability P br as a function of the lifetime of the atomic ground stateτ1S for the combined94and98µm thick Ni targets.The experimentally determined P br with statistical and total errors translates into a value of the lifetime with corresponding errors.157AcknowledgementsWe are indebted to the CERN PS crew for providing a beam of excellent qual-ity.This work was supported by CERN,the Grant Agency of the Czech Repub-lic,grant No.202/01/0779and202/04/0793,the Greek General Secretariat of Re-search and Technology(Greece),the University of Ioannina Research Committee (Greece),the IN2P3(France),the Istituto Nazionale di Fisica Nucleare(Italy),the Grant-in-Aid for Scientific Research from Japan Society for the Promotion of Sci-ence07454056,08044098,09640376,09440012,11440082,11640293,11694099, 12440069,14340079,and15340205,the Ministry of Education and Research,un-der project CORINT No.1/2004(Romania),the Ministery of Industry,Science and Technologies of the Russian Federation and the Russian Foundation for Basic Re-search(Russia),under project01-02-17756,the Swiss National Science Founda-tion,the Ministerio de Ciencia y Tecnologia(Spain),under projects AEN96-1671 and AEN99-0488,the PGIDT of Xunta de Galicia(Spain).References[1] B.Adeva et al.,DIRAC proposal,CERN/SPSLC95-1,SPSLC/P284(1995).[2]J.Uretsky and J.Palfrey,Phys.Rev.121(1961)1798.[3]H.-W.Hammer and J.N.Ng,Eur.Phys.J.A6(1999)115.[4]S.Deser et al.,Phys.Rev.96(1954)774.[5]S.M.Bilenky et al.,Yad.Phys.10(1969)812;(Sov.J.Nucl.Phys.10(1969)469).[6]H.Jallouli and H.Sazdjian,Phys.Rev.D58(1998)014011;Erratum:ibid.,D58(1998)099901.[7]M.A.Ivanov et al.Phys.Rev.D58(1998)094024.[8]J.Gasser et al.,Phys.Rev.D64(2001)016008;hep-ph/0103157.[9] A.Gashi et al.,Nucl.Phys.A699(2002)732.[10]S.Weinberg,Physica A96(1979)327;J.Gasser and H.Leutwyler,Phys.Lett.B125(1983)325;ibid Nucl.Phys.B250465,517,539.[11]G.Colangelo,J.Gasser and H.Leutwyler,Nucl.Phys.B603(2001)125.[12]M.Knecht et al.,Nucl.Phys.B457(1995)513.[13]L.Rosselet et al.,Phys.Rev.D15(1977)547.[14]S.Pislak et al.,Phys.Rev.Lett,87(2001)221801.[15]L.L.Nemenov,Yad.Fiz.41(1985)980;(Sov.J.Nucl.Phys.41(1985)629).16。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :h e p -e x /0307068v 2 4 N o v 2003DESY–03–094July 2003Measurement of the open-charm contribution to the diffractive proton structure function ZEUS Collaboration Abstract Production of D ∗±(2010)mesons in diffractive deep inelastic scattering has beenmeasured with the ZEUS detector at HERA using an integrated luminosity of 82pb −1.Diffractive events were identified by the presence of a large rapidity gap in the final state.Differential cross sections have been measured in the kinematic region 1.5<Q 2<200GeV 2,0.02<y <0.7,x I P <0.035,β<0.8,p T (D ∗±)>1.5GeV and |η(D ∗±)|<1.5.The measured cross sections are compared to theoretical predictions.The results are presented in terms of the open-charm contribution to the diffractive proton structure function.The data demonstrate a strong sensitivity to the diffractive parton densities.The ZEUS CollaborationS.Chekanov,M.Derrick,D.Krakauer,J.H.Loizides1,S.Magill,B.Musgrave,J.Repond, R.YoshidaArgonne National Laboratory,Argonne,Illinois60439-4815nM.C.K.MattinglyAndrews University,Berrien Springs,Michigan49104-0380P.Antonioli,G.Bari,M.Basile,L.Bellagamba,D.Boscherini,A.Bruni,G.Bruni, G.Cara Romeo,L.Cifarelli,F.Cindolo,A.Contin,M.Corradi,S.De Pasquale,P.Giusti, G.Iacobucci,A.Margotti,R.Nania,F.Palmonari,A.Pesci,G.Sartorelli,A.Zichichi University and INFN Bologna,Bologna,Italy eG.Aghuzumtsyan,D.Bartsch,I.Brock,S.Goers,H.Hartmann,E.Hilger,P.Irrgang,H.-P.Jakob,A.Kappes2,U.F.Katz2,O.Kind,U.Meyer,E.Paul3,J.Rautenberg, R.Renner,A.Stifutkin,J.Tandler,K.C.Voss,M.Wang,A.Weber4Physikalisches Institut der Universit¨a t Bonn,Bonn,Germany bD.S.Bailey5,N.H.Brook5,J.E.Cole,B.Foster,G.P.Heath,H.F.Heath,S.Robins,E.Rodrigues6,J.Scott,R.J.Tapper,M.WingH.H.Wills Physics Laboratory,University of Bristol,Bristol,United Kingdom mM.Capua,A.Mastroberardino,M.Schioppa,G.SusinnoCalabria University,Physics Department and INFN,Cosenza,Italy eJ.Y.Kim,Y.K.Kim,J.H.Lee,I.T.Lim,M.Y.Pac7Chonnam National University,Kwangju,Korea gA.Caldwell8,M.Helbich,X.Liu,B.Mellado,Y.Ning,S.Paganis,Z.Ren,W.B.Schmidke, F.SciulliNevis Laboratories,Columbia University,Irvington on Hudson,New York10027oJ.Chwastowski,A.Eskreys,J.Figiel,K.Olkiewicz,P.Stopa,L.ZawiejskiInstitute of Nuclear Physics,Cracow,Poland iL.Adamczyk,T.Bo l d,I.Grabowska-Bo l d,D.Kisielewska, A.M.Kowal,M.Kowal, T.Kowalski,M.Przybycie´n,L.Suszycki,D.Szuba,J.Szuba9Faculty of Physics and Nuclear Techniques,University of Mining and Metallurgy,Cracow, Poland pA.Kota´n ski10,W.S l omi´n ski11Department of Physics,Jagellonian University,Cracow,PolandIV.Adler,L.A.T.Bauerdick12,U.Behrens,I.Bloch,K.Borras,V.Chiochia,D.Dannheim, G.Drews,J.Fourletova,U.Fricke,A.Geiser,F.Goebel8,P.G¨o ttlicher13,O.Gutsche, T.Haas,W.Hain,G.F.Hartner,S.Hillert,B.Kahle,U.K¨o tz,H.Kowalski14,G.Kram-berger,bes,D.Lelas,B.L¨o hr,R.Mankel,I.-A.Melzer-Pellmann,M.Moritz15, C.N.Nguyen,D.Notz,M.C.Petrucci16,A.Polini,A.Raval,U.Schneekloth,F.Selonke3, U.Stoesslein,H.Wessoleck,G.Wolf,C.Youngman,W.ZeunerDeutsches Elektronen-Synchrotron DESY,Hamburg,GermanyS.SchlenstedtDESY Zeuthen,Zeuthen,GermanyG.Barbagli,E.Gallo,C.Genta,P.G.PelferUniversity and INFN,Florence,Italy eA.Bamberger,A.Benen,N.CoppolaFakult¨a t f¨u r Physik der Universit¨a t Freiburg i.Br.,Freiburg i.Br.,Germany bM.Bell,P.J.Bussey,A.T.Doyle,C.Glasman,J.Hamilton,S.Hanlon,S.W.Lee,A.Lupi, D.H.Saxon,I.O.SkillicornDepartment of Physics and Astronomy,University of Glasgow,Glasgow,United King-dom mI.GialasDepartment of Engineering in Management and Finance,Univ.of Aegean,GreeceB.Bodmann,T.Carli,U.Holm,K.Klimek,N.Krumnack,E.Lohrmann,ite, H.Salehi,S.Stonjek17,K.Wick,A.Ziegler,Ar.ZieglerHamburg University,Institute of Exp.Physics,Hamburg,Germany bC.Collins-Tooth,C.Foudas,R.Gon¸c alo6,K.R.Long,A.D.TapperImperial College London,High Energy Nuclear Physics Group,London,United King-dom mP.Cloth,D.FilgesForschungszentrum J¨u lich,Institut f¨u r Kernphysik,J¨u lich,GermanyK.Nagano,K.Tokushuku18,S.Yamada,Y.YamazakiInstitute of Particle and Nuclear Studies,KEK,Tsukuba,Japan fA.N.Barakbaev,E.G.Boos,N.S.Pokrovskiy,B.O.ZhautykovInstitute of Physics and Technology of Ministry of Education and Science of Kazakhstan, Almaty,KazakhstanH.Lim,D.SonKyungpook National University,Taegu,Korea gIIK.PiotrzkowskiInstitut de Physique Nucl´e aire,Universit´e Catholique de Louvain,Louvain-la-Neuve,Bel-giumF.Barreiro,O.Gonz´a lez,barga,J.del Peso,E.Tassi,J.Terr´o n,M.V´a zquez Departamento de F´ısica Te´o rica,Universidad Aut´o noma de Madrid,Madrid,Spain l M.Barbi,F.Corriveau,S.Gliga,inesse,S.Padhi,D.G.StairsDepartment of Physics,McGill University,Montr´e al,Qu´e bec,Canada H3A2T8aT.TsurugaiMeiji Gakuin University,Faculty of General Education,Yokohama,Japan fA.Antonov,P.Danilov,B.A.Dolgoshein,D.Gladkov,V.Sosnovtsev,S.Suchkov Moscow Engineering Physics Institute,Moscow,Russia jR.K.Dementiev,P.F.Ermolov,Yu.A.Golubkov,I.I.Katkov,L.A.Khein,I.A.Korzhav-ina,V.A.Kuzmin,B.B.Levchenko19,O.Yu.Lukina,A.S.Proskuryakov,L.M.Shcheglova, N.N.Vlasov,S.A.ZotkinMoscow State University,Institute of Nuclear Physics,Moscow,Russia kN.Coppola,S.Grijpink,E.Koffeman,P.Kooijman,E.Maddox,A.Pellegrino,S.Schagen, H.Tiecke,J.J.Velthuis,L.Wiggers,E.de WolfNIKHEF and University of Amsterdam,Amsterdam,Netherlands hN.Br¨u mmer,B.Bylsma,L.S.Durkin,T.Y.LingPhysics Department,Ohio State University,Columbus,Ohio43210nA.M.Cooper-Sarkar,A.Cottrell,R.C.E.Devenish,J.Ferrando,G.Grzelak,S.Patel, M.R.Sutton,R.WalczakDepartment of Physics,University of Oxford,Oxford United Kingdom mA.Bertolin,R.Brugnera,R.Carlin,F.Dal Corso,S.Dusini,A.Garfagnini,S.Limentani, A.Longhin,A.Parenti,M.Posocco,L.Stanco,M.TurcatoDipartimento di Fisica dell’Universit`a and INFN,Padova,Italy eE.A.Heaphy,F.Metlica,B.Y.Oh,J.J.Whitmore20Department of Physics,Pennsylvania State University,University Park,Pennsylvania 16802oY.IgaPolytechnic University,Sagamihara,Japan fG.D’Agostini,G.Marini,A.NigroDipartimento di Fisica,Universit`a’La Sapienza’and INFN,Rome,Italy eIIIC.Cormack21,J.C.Hart,N.A.McCubbinRutherford Appleton Laboratory,Chilton,Didcot,Oxon,United Kingdom mC.HeuschUniversity of California,Santa Cruz,California95064nI.H.ParkDepartment of Physics,Ewha Womans University,Seoul,KoreaN.PavelFachbereich Physik der Universit¨a t-Gesamthochschule Siegen,GermanyH.Abramowicz,A.Gabareen,S.Kananov,A.Kreisel,A.LevyRaymond and Beverly Sackler Faculty of Exact Sciences,School of Physics,Tel-Aviv University,Tel-Aviv,Israel dM.KuzeDepartment of Physics,Tokyo Institute of Technology,Tokyo,Japan fT.Abe,T.Fusayasu,S.Kagawa,T.Kohno,T.Tawara,T.YamashitaDepartment of Physics,University of Tokyo,Tokyo,Japan fR.Hamatsu,T.Hirose3,M.Inuzuka,S.Kitamura22,K.Matsuzawa,T.Nishimura Tokyo Metropolitan University,Department of Physics,Tokyo,Japan fM.Arneodo23,M.I.Ferrero,V.Monaco,M.Ruspa,R.Sacchi,A.SolanoUniversit`a di Torino,Dipartimento di Fisica Sperimentale and INFN,Torino,Italy e T.Koop,G.M.Levman,J.F.Martin,A.MireaDepartment of Physics,University of Toronto,Toronto,Ontario,Canada M5S1A7a J.M.Butterworth,C.Gwenlan,R.Hall-Wilton,T.W.Jones,M.S.Lightwood,B.J.West Physics and Astronomy Department,University College London,London,United King-dom mJ.Ciborowski24,R.Ciesielski25,R.J.Nowak,J.M.Pawlak,J.Sztuk26,T.Tymieniecka27, leja27,leja,A.F.˙ZarneckiWarsaw University,Institute of Experimental Physics,Warsaw,Poland qM.Adamus,P.PlucinskiInstitute for Nuclear Studies,Warsaw,Poland qY.Eisenberg,L.K.Gladilin28,D.Hochman,U.Karshon,M.RivelineDepartment of Particle Physics,Weizmann Institute,Rehovot,Israel cD.K¸c ira,mmers,L.Li,D.D.Reeder,A.A.Savin,W.H.SmithDepartment of Physics,University of Wisconsin,Madison,Wisconsin53706nIVA.Deshpande,S.Dhawan,P.B.StraubDepartment of Physics,Yale University,New Haven,Connecticut06520-8121nS.Bhadra,C.D.Catterall,S.Fourletov,G.Hartner,S.Menary,M.Soares,J.Standage Department of Physics,York University,Ontario,Canada M3J1P3aV1also affiliated with University College London2on leave of absence at University of Erlangen-N¨u rnberg,Germany3retired4self-employed5PPARC Advanced fellow6supported by the Portuguese Foundation for Science and Technology(FCT)7now at Dongshin University,Naju,Korea8now at Max-Planck-Institut f¨u r Physik,M¨u nchen/Germany9partly supported by the Israel Science Foundation and the Israel Ministry of Science 10supported by the Polish State Committee for Scientific Research,grant no.2P03B 0932211member of Dept.of Computer Science12now at Fermilab,Batavia/IL,USA13now at DESY group FEB14on leave of absence at Columbia Univ.,Nevis Labs.,N.Y./USA15now at CERN16now at INFN Perugia,Perugia,Italy17now at Univ.of Oxford,Oxford/UK18also at University of Tokyo19partly supported by the Russian Foundation for Basic Research,grant02-02-81023 20on leave of absence at The National Science Foundation,Arlington,VA/USA21now at Univ.of London,Queen Mary College,London,UK22present address:Tokyo Metropolitan University of Health Sciences,Tokyo116-8551, Japan23also at Universit`a del Piemonte Orientale,Novara,Italy24also at L´o d´z University,Poland25supported by the Polish State Committee for Scientific Research,grant no.2P03B 0722226 L´o d´z University,Poland27supported by German Federal Ministry for Education and Research(BMBF),POL 01/04328on leave from MSU,partly supported by University of Wisconsin via the U.S.-Israel BSFVIa supported by the Natural Sciences and Engineering Research Council ofCanada(NSERC)b supported by the German Federal Ministry for Education and Research(BMBF),under contract numbers HZ1GUA2,HZ1GUB0,HZ1PDA5, HZ1VFA5c supported by the MINERVA Gesellschaft f¨u r Forschung GmbH,the IsraelScience Foundation,the U.S.-Israel Binational Science Foundation and the Benozyio Center for High Energy Physicsd supported by the German-Israeli Foundation and the Israel Science Foundatione supported by the Italian National Institute for Nuclear Physics(INFN)f supported by the Japanese Ministry of Education,Culture,Sports,Scienceand Technology(MEXT)and its grants for Scientific Researchg supported by the Korean Ministry of Education and Korea Science and Engi-neering Foundationh supported by the Netherlands Foundation for Research on Matter(FOM)i supported by the Polish State Committee for Scientific Research,grant no.620/E-77/SPUB-M/DESY/P-03/DZ247/2000-2002j partially supported by the German Federal Ministry for Education and Re-search(BMBF)k supported by the Fund for Fundamental Research of Russian Ministry for Science and Education and by the German Federal Ministry for Education and Research(BMBF)l supported by the Spanish Ministry of Education and Science through funds provided by CICYTm supported by the Particle Physics and Astronomy Research Council,UKn supported by the US Department of Energyo supported by the US National Science Foundationp supported by the Polish State Committee for Scientific Research,grant no.112/E-356/SPUB-M/DESY/P-03/DZ301/2000-2002,2P03B13922q supported by the Polish State Committee for Scientific Research,grant no.115/E-343/SPUB-M/DESY/P-03/DZ121/2001-2002,2P03B07022VII1IntroductionIn ep deep inelastic scattering(DIS)at HERA,final-state hadrons are dominantly pro-duced by interactions between virtual photons and incoming protons.Diffractive interac-tions,characterized by a large rapidity gap in the distribution of thefinal-state hadrons, have been observed and extensively studied at HERA[1,2,3,4,5,6,7,8,9].The measure-ments of the diffractive DIS cross sections[2,3,4,5,7]have been quantified in terms of a diffractive structure function,F D2,defined in analogy with the proton structure function, F2.The diffractive parton densities,determined from these measurements,are domi-nated by gluons.The diffractive process at HERA has often been considered to proceed through the exchange of an object carrying the quantum numbers of the vacuum,called the Pomeron(I P).In the resolved-Pomeron model[10],the exchanged Pomeron acts as a source of partons,one of which interacts with the virtual photon.In an alternative view, the diffractive process at HERA can be described by the dissociation of the virtual photon into a q¯q or q¯q g state which interacts with the proton by the exchange of two gluons or, more generally,a gluon ladder with the quantum numbers of the vacuum[11,12,13]. Charm production in diffractive DIS,which has also been measured by the H1and ZEUS collaborations[14,15],allows quantitative tests of the models due to the sensi-tivity of charm production to gluon-initiated processes[16].Calculations based on a gluon-dominated resolved Pomeron predict a large charm rate in diffractive DIS[17,18]. In the two-gluon-exchange models,the rate from the q¯q g state is similar to that predicted by the resolved-Pomeron model,while the rate from the q¯q state is lower.In this analysis,charm production,tagged using D∗±mesons,is studied in diffractive interactions identified by the presence of a large rapidity gap between the proton at high rapidities and the centrally-produced hadronic system.The luminosity for the present measurement is about two times larger than in the previous ZEUS study[15].The increase in luminosity and an improved rapidity acceptance in the proton direction allow a more detailed comparison with the model predictions in a wider kinematic range.The open-charm contribution to the diffractive proton structure function is measured for thefirst time.2Experimental set-upThe analysis was performed with data taken from1998to2000,when HERA collided electrons or positrons1with energy E e=27.5GeV with protons of energy E p=920GeVyielding a centre-of-mass energy of318GeV.The results are based on the sum of thee−p and e+p samples,corresponding to integrated luminosities of16.4±0.3pb−1and65.3±1.5pb−1,respectively.A detailed description of the ZEUS detector can be found elsewhere[19].A brief out-line of the components that are most relevant for this analysis is given below.Charged particles are tracked in the central tracking detector(CTD)[20],which operates in a magneticfield of1.43T provided by a thin superconducting solenoid.The CTD consists of72cylindrical drift chamber layers,organised in nine superlayers covering the polar-angle2region15◦<θ<164◦.The transverse-momentum resolution for full-length tracks isσ(p T)/p T=0.0058p T⊕0.0065⊕0.0014/p T,with p T in GeV.The high-resolution uranium–scintillator calorimeter(CAL)[21]consists of three parts: the forward(FCAL),the barrel(BCAL)and the rear(RCAL)calorimeters.Each part is subdivided transversely into towers and longitudinally into one electromagnetic sec-tion(EMC)and either one(in RCAL)or two(in BCAL and FCAL)hadronic sections (HAC).The smallest subdivision of the calorimeter is called a cell.The CAL energy res-√olutions,as measured under test-beam conditions,areσ(E)/E=0.18/E for hadrons,with E in GeV.The timing resolution of the CAL is better than1ns for energy deposits greater than4.5GeV.In1998-2000,the forward plug calorimeter(FPC)[22]was installed in the20×20cm2 beam hole of the FCAL,with a small hole of radius3.15cm in the centre to accom-modate the beam pipe.The FPC increased the forward calorimetric coverage by about 1unit of pseudorapidity toη≤5.The FPC consisted of a lead–scintillator sandwich calorimeter divided longitudinally into electromagnetic and hadronic sections that wereread out separately by wavelength-shiftingfibers and photomultipliers.The energy res-√olution,as measured under test-beam conditions,wasσ(E)/E=0.41/E⊕0.06for electrons and pions,respectively,with E in GeV.The position of electrons scattered at a small angle with respect to the electron beam direction was measured using the small-angle rear tracking detector(SRTD)[23].The luminosity was determined from the rate of the bremsstrahlung process ep→eγp,where the photon was measured with a lead–scintillator calorimeter[24]located at Z=−107m.2 ,where the polar angle,θ,is measured with respect to the proton beam direction.23Kinematics and reconstruction of variablesThe four-momenta k ,k ′and P label the incoming electron,outgoing electron and the incoming proton,respectively,in DIS events:e (k )+p (P )→e (k ′)+anything .To describe the kinematics of DIS events,any two of the following invariants can be used:Q 2=−q 2=−(k −k ′)2;x =Q 2P ·k ;W 2=Q 2(1−x )Q 2+W 2,where M X is the invariant mass of the system X .This variable is the fraction of the incoming proton momentum carried by the diffractive exchange;•β=x Q 2+M 2X .In an interpretation in which partonic structure is ascribed to the diffractive exchange,βis the longitudinal momentum fraction of the exchange that is carried by the struck quark.The above expressions neglect the proton mass.The square of the four-momentum trans-fer at the proton vertex,t ,was not measured;thus all results are implicitly integrated over this variable,which was assumed to be zero in the expressions for x I P and β.The mass of the diffractive system X was calculated from EFOs using:M 2X = i E i 2− i P X,i 2− i P Y,i 2− i P Z,i 2,where the sum i runs over the EFOs not associated with the scattered electron.The process studied in this paper is ep →eXp →e (D ∗±X ′)p ,in which the system X includes at least one D ∗±meson.The latter was reconstructed using the mass-differencemethod[28]in the decay channel D ∗+→D 0π+s followed by D 0→K −π+(+c.c.),where3πs indicates the“slow”pion.The fractional momentum of the D∗±in the photon-proton system is defined as2|p∗(D∗±)|x(D∗±)=Q2+4m2c as in[18].The Peter-son fragmentation function(withǫ=0.035[34])was used for the charm decay.The probability for charm to fragment into a D∗±meson was set to f(c→D∗+)=0.235[35]. The two-gluon-exchange models considerfluctuations of the virtual photon into q¯q or q¯q g colour dipoles that interact with the proton via colour-singlet exchange;the simplest form of which is a pair of gluons[36].The virtual-photonfluctuations into c¯c(Fig.1b)4and c¯c g states(Fig.1c)can lead to diffractive open-charm production.At high xI Pval-ues,quark exchanges are expected to become significant.Thus,the two-gluon-exchangecalculations are expected to be valid only at low xI P values(xI P<0.01).In recent calcu-lations[37,12,38,39],the cross section for two-gluon exchange is related to the square of the unintegrated gluon distribution of the proton which depends on the gluon transverse momentum,k T,relative to the proton direction.In the“saturation”model[39,40],the calculation of the q¯q g cross section is performed under the assumption of strong k T order-ing of thefinal-state partons,which corresponds to k(g)T≪k(q,¯q)T.The parameters of the model were tuned to describe the total photon-proton cross section measured at HERA. Alternatively,in the model of Bartels et al.[37,12,38],configurations without strong k T ordering are included in the q¯q g cross-section calculation and the minimum value for the final-state-gluon transverse momentum,k cut T,g,is a free parameter.The sum of the c¯c and c¯c g contributions in the saturation model and the model of Bartels et al.are hereafter re-ferred to as SATRAP and BJLW,respectively.Both the SATRAP and BJLW predictions were calculated using the MC generator RAPGAP2.08/06[41],the proton PDF param-eterisation GRV94HO[42],m c=1.45GeV andµR=µF=corrections were included in the simulation although their effects were negligible.The c¯c and c¯c g components were generated separately and later combined in the proportion 16%:84%which provided the best description of theβdistribution of the data.The RAPGAP MC sample was used to evaluate the acceptance.Three MC samples were used to estimate the model dependence of the acceptance corrections:the RIDI MC sample,a sample generated with RAPGAP using parton showers as implemented in LEPTO6.1[48]to simulate the higher-order QCD corrections,and a sample generated with RAPGAP using the Pomeron PDF parameterisation“H1fit3”[14].To estimate the non-diffractive DIS background and to measure the ratio of diffractive to inclusive D∗±production(see Section8.2),two MC generators were used:RAPGAP in the non-diffractive mode for the nominal calculations and HERWIG6.301[49]as a systematic check.The RAPGAP parameters used were the same as those used in the ZEUS measurement of the inclusive DIS D∗±cross sections[50].To generate charm pro-duction via the leading-order BGF process with HERWIG,the CTEQ5L[51]proton PDF parameterisation and m c=1.5GeV were used.Hadronisation in HERWIG is simulated with a cluster algorithm[52].In this analysis,thefinal-state proton was not detected.To estimate and subtract the contribution from the diffractive processes where the proton dissociates into a system N, ep→eXN→e(D∗±X′)N,four MC generators were used:DIFFVM[53]for the nominal calculations and RAPGAP,PHOJET[54]and EPSOFT2.0[55]for systematic checks. The DIFFVM MC program provides a detailed description of the proton-dissociativefi-nal state.The mass spectrum,M N,of the system N is generated as a superposition of.The default N∗+resonances and a continuum having the form dσ/dM2N∝M−2(1+ǫ)Nparameter valueǫ=0.0808[56]was used.In the RAPGAP simulation of proton dis-sociation,the proton splits into a quark and di-quark and the Pomeron is assumed to couple only to the single quark.The M N spectrum follows a1/M N distribution.In PHO-JET,M N is calculated from the triple-Pomeron kinematics[54]and an approximation of the low-mass-resonance structure.In EPSOFT,the M N-spectrum generation relies on a parameterisation of the pp→pN data.The generated events were passed through the GEANT-based[57]simulation of the ZEUS detector and trigger.They were reconstructed by the same program chain as the data.66Event selection and D∗±reconstruction6.1Trigger and DIS selectionEvents were selected online with a three-level trigger[19,58].At thefirst level,events with an electron candidate in the EMC sections of RCAL or BCAL were selected[59].In the latter case,a coincidence with a track originating at the nominal interaction point was required.At the second level,the non-ep background was further reduced by removing events with CAL timing inconsistent with an ep interaction.At the third level,events were fully reconstructed and selected by requiring a coincidence of a scattered-electron candidate found within the CAL and a D∗±candidate reconstructed in the nominal de-cay mode using charged tracks measured by the CTD.The requirements were similar to,but looser than,the offline cuts described below.The efficiency of the online D∗±reconstruction,determined relative to an inclusive DIS trigger,was above95%.The following criteria were applied offline to select DIS events:•an electron with energy above10GeV;•the impact point of the scattered electron on the RCAL lies outside the region26×14 cm2centred on the beamline;•40<δ<65GeV,whereδ= i(E i−P Z,i)and the sum runs over the EFOs from the hadronic system and the energy deposited by the identified electron;•a vertex position|Z vtx|<50cm.The events were restricted to the kinematic region1.5<Q2<200GeV2and0.02<y< 0.7.6.2D∗±reconstructionCharged tracks with p T>0.12GeV and|η|<1.75were selected.Only tracks assigned to the primary event vertex and with hits in at least three superlayers of the CTD were considered.Two oppositely charged tracks,each with p T>0.5GeV,were combined to form a D0candidate.The tracks were alternately assigned the mass of a charged kaon and a charged pion and the invariant mass of the track pair,M(Kπ),was calculated. Only D0candidates that satisfy1.81<M(Kπ)<1.92GeV were kept.Any additional track,with p T>0.12GeV and charge opposite to that of the kaon track,was assigned the pion mass and combined with the D0candidate to form a D∗±candidate with invariant mass M(Kππs).The D∗±candidates were required to have p T(D∗)>1.5GeV and |η(D∗)|<1.5.7In the distribution of the mass difference,∆M=M(Kππs)−M(Kπ),for selected D∗±candidates,a clear signal at the nominal value of M(D∗±)−M(D0)was observed(not shown).The combinatorial background under this signal was estimated from the mass-difference distribution for wrong-charge combinations,in which both tracks forming the D0candidates have the same charge and the third track has the opposite charge.The number of reconstructed D∗±mesons was determined by subtracting the wrong-charge ∆M distribution after normalising it to the∆M distribution of D∗±candidates with the appropriate charges in the range0.15<∆M<0.17GeV.The subtraction,performed in the range0.1435<∆M<0.1475GeV,yielded an inclusive signal of4976±103D∗±mesons.6.3Selection of diffractive eventsDiffractive events are characterised by the presence of a large rapidity gap between the proton at high rapidities and the centrally-produced hadronic system.To select such events,the following two requirements were applied:•E FPC<1.5GeV,where E FPC is the energy deposited in the FPC;•ηmax<3,whereηmax is the pseudorapidity of the most-forward EFO measured without using FPC information and with energy above400MeV.This selection is illustrated in Fig.2,where the distribution ofηmax is shown for D∗±mesons obtained after the wrong-charge-background subtraction.The data are compared to theηmax distributions of the non-diffractive RAPGAP and HERWIG MC samples and to the sum of the non-diffractive and diffractive RAPGAP MC.In Fig.2a,the distributions are shown for events with any E FPC value.The large peak atηmax∼3.5 corresponds to non-diffractive events in which the proton remnant deposits energy around the beam direction.On the low side of the peak,the contribution from non-diffractive interactions exhibits an exponential fall-off,leaving an excess at low values ofηmax which is populated predominantly by diffractive events.Figure2b shows that the requirement E FPC<1.5GeV strongly suppresses the contribution from non-diffractive interactions. Requiringηmax<3in addition reduces the remaining non-diffractive background and ensures a gap of at least two units of pseudorapidity with respect to the edge of the forward calorimetric coverage(see Section2).The selected events were analysed in terms of the diffractive variables x,βand M X.ToI Paccount for the restriction imposed by theηmax<3requirement,a cut of x<0.035wasI Papplied.In addition,a cut ofβ<0.8was also used because diffractive charm production in DIS is strongly suppressed at largeβvalues due the dominant contribution of events with small Q2and large M X values.8Figure3shows the∆M distribution after the above cuts.The number of D∗±after the wrong-charge-background subtraction is253±21.Figure4shows the number of reconstructed D∗±mesons in bins of the variables p T(D∗±),,log(M2X),log(Q2)and W.The data are compared to the diffractive η(D∗±),x(D∗±),β,xI PRAPGAP and RIDI simulations(normalised to the data).Both simulations reproduce the shapes of the data.6.4Subtraction of the proton-dissociative contributionDiffractive events with proton dissociation can pass the E FPC<1.5GeV andηmax<3 requirements if the major part of the proton-dissociative system escapes undetected down the forward beampipe.The proton-dissociative contribution was determined from the distribution of E FPC for events selected with relaxed D∗±reconstruction cuts and with-out cutting on E FPC.To ensure a gap of at least two units of pseudorapidity between the proton-dissociative system,tagged by the FPC,and the system X,a requirement of ηmax<1.75was applied.Figure5compares the E FPC distribution for these events to the distributions of the diffractive RAPGAP and proton-dissociative DIFFVM MC samples. The MC samples were combined in the proportion providing the best description of the E FPC distribution,and their sum was normalised to the ing the normalisation factors obtained for the two MC samples,the proton-dissociative contribution was calcu-lated for the nominal diffractive selection described in Section6.3.The proton-dissociative contribution was determined to be16%with negligible statistical uncertainty;the sys-tematic uncertainty was obtained as follows,where the effects of each source are shown in parentheses:•the parameter b,regulating the shape of the M N continuum distribution in the DIF-FVM MC simulation,was varied between0.7and1.5(+3.7−3.0%);•uncertainties in the low-mass resonance structure and other details of the simulation of the proton-dissociative system were estimated by using the PHOJET,RAPGAPand EPSOFT MC generators(+1.6−0.9%);•a shift of±10%due to the FPC energy-scale uncertainty(+0.5−0.1%);•a larger area,including the FPC and neighbouring FCAL towers,was used to tag the proton-dissociative system(−2.7%).This check is sensitive to the high-M N proton-dissociative contribution and to details of the FPC and FCAL simulation.These systematic uncertainties were added in quadrature separately for the positive and negative variations to determine the overall systematic uncertainty of±4.1%.The proton-dissociative contribution of(16±4)%was assumed to be independent of all kinematic variables and was subtracted from all measured cross sections.9。
