Solvent coarse-graining and the string method applied to the hydrophobic collapse of a hydr

合集下载

计算材料学逾渗理论

转变
堵塞/流通抑制/流行断开/联结绝缘体/金属导体正常导电/超导绝缘体/金属导体非传播/传播禁闭/非禁闭正常的/超流的绝缘体/金属导体顺磁性的/铁磁体的液体/凝胶液体/玻璃局域态/扩展态类似于电阻网络
3.1 逾渗的基本理论
逾渗理论应用如此广泛，其主要原因是自然界中广泛地存在着无序和随机结构。随着结构联结程度或某些参数，诸如某种密度、占据数等的突然增加出现长程联结．这就使逾渗理论成为描述这些现象的自然模型。另一方面，逾渗理论不要求精深的数学能力，却可以为空间随机过程提供一个明确、清晰、直观的描述。
对于键逾渗过程，每条键或者是联结的，或者是不联结的；联结的百分率为p，不联结的百分率为1-p。应该指出，这儿必须假定系统是完全无序的，意即每条键的联结概率与其相邻键的逾渗的基本理论
对于座逾渗，每条键都是联结的，但“座”具有结构的无规联结性特征∶每一个座或者是联结的（畅通的），或者是不联结的（堵塞的），相应的百分率分别为p和1-p。仍假定，对于每一个座，概率不受其相邻点的状态的影响。
L=150情况下，不同的概率p下团簇的尺度分布情况。
p=0.58的情况下，它的尺度分布密度函数可以拟合为p(x)=0.37*x-1.72
3.2 逾渗阀值的计算
团簇的分形特征
处于临界状态附近的渗流系统中的大的团簇基本上都是具有自相似的分形体。
通过Box Covering （盒覆盖）方法来计算这个红色大团块的分形维。
ps' f ps
p(sn1) p(sn )4 4p(s n )3 (1- p(sn )) 2p(s n )2 (1- p(sn ))2
p(sn)的四次方项对应的是规则中的最后一个规则（也就是说在原始尺度下，黑格要连续出现4次，它的概率显然是p(sn)4），3次方项是规则左边有三个黑格的情况，这一共有4条规则，所以系数为4，概率是黑格连续出现3次，并且最后一次是白格，所以是p(sn)3(1-p(sn))；2次方相对应的是两种两个黑色竖向连在一起的2条规则，系数为2.

密度泛函理论

1. Lennard-Jones （LJ）势
➢ 最常用旳描述原子间范德华力旳经验势。最广泛使用旳是 12-6 LJ：
V (r)
4
12
r12
6
r6
F(r)
V
r
24
r
12
2 r12
6
r6
rˆ
➢ 惰性气体旳原子间相互作用仅用 LJ 就基本能够完全描述。
➢ 截断距离（cutoff distance）：对于短程作用，不小于 cutoff 旳贡献是常数。
➢ Glue Model
只合用于单一金属。很好地平衡了表面和内部旳构造和能量。
Vi
rij
U
rij
ji
j
3. 化学和生物体系旳力场
➢ 成键作用（Bonded Interactions）：Bonds，Valence Angles，Dihedral Angles (Torsional Angles), Improper Dihedral Angles ➢ 非成键作用（Nonbonded Interactions）：范德华力和静电力
➢ 困难在于全原子层面上，原子间相互作用并不集中在局部。而在第一性层面上，电子及其相互作用基本局限在相应旳原子核周围。
➢ 不同旳粗粒化措施着重于重建不同旳物性，如构造或扩散特征等。
➢ 某些粗粒化措施假定作用势旳函数形式，然后用全原子模拟旳成果定参数。另一类从构造函数（RDF）出发，反推出作用势。我们旳措施从全原子作用势出发，经过数学变换较严格地得到粗粒化力场。
P86 c
LDA c
P c
86
P86 c
eC f 7/3
2
a
C C 7/6
f 21/3

jade微观应变计算

jade微观应变计算英文回答：Jade, known for its toughness, is a type of ornamental stone. Understanding its mechanical behavior can help in assessing its durability and fracture resistance. Strain is a measure of deformation, which is a key factor in assessing a material's mechanical properties. Here's a detailed explanation of how jade microstrain is calculated:1. Sample Preparation: Thin sections of jade are carefully prepared for microscopic examination. The thickness of the sample should be appropriate for the resolution of the microscope being used.2. Microscopic Imaging: The prepared sample is placed under a polarized light microscope to capture high-resolution images. These images provide detailed information about the microstructure of the jade, including grain boundaries, cracks, and other defects.3. Image Analysis: Specialized software is used to analyze the microscopic images. This software can measure the grain size, quantify the porosity, and identify any defects present in the jade sample.4. Strain Calculation: The strain in the jade sample can be calculated by measuring the displacement of grains or other features within the microstructure. This is typically done by comparing the original image to an image taken after a controlled deformation experiment.5. Strain Mapping: Strain mapping techniques can be used to visualize the spatial distribution of strain within the jade sample. This provides valuable information about localized areas of stress concentration or deformation.The calculation of jade microstrain involves meticulous sample preparation, high-resolution imaging, and advanced image analysis techniques. By accurately determining the strain distribution, researchers and engineers can better understand the mechanical behavior of jade and predict itsperformance under various loading conditions.中文回答：玉是一种以坚韧著称的观赏石，了解其力学行为有助于评估其耐用性和抗断性。

石油缩写

calcarenite calcarenite calcareous calcilutite calcilutite calcirudite calcisilttite calcisilttite calcite calcium calcium chloride calculate calculate caliper caliper cambrian cambrian carbide carbide carbon tetrachloride carbonaceous carbonaceous carbonate carboniferous carboniferous carboniferous casing casing casing shoe cading size caved caved cavern cavern cavernous cavernous cavings cemented cemented cenozoic centigrade centigrade degree centimetre cephalopoda chalcedony chalk chalky channel charophyta check shots checked chert cherty chitin chitin
4
219 220 221 222 223 224 225 226 227 228 229 230 231 232 233 234 235 236 237 238 239 240 241 242 243 244 245 246 247 248 249 250 251 252 253 254 255 256 257 258 259 260 261 262 263 264 265 266 267 268 269 270 271 272 273

应用地球化学元素丰度数据手册-原版

应用地球化学元素丰度数据手册迟清华鄢明才编著地质出版社·北京·1内容提要本书汇编了国内外不同研究者提出的火成岩、沉积岩、变质岩、土壤、水系沉积物、泛滥平原沉积物、浅海沉积物和大陆地壳的化学组成与元素丰度，同时列出了勘查地球化学和环境地球化学研究中常用的中国主要地球化学标准物质的标准值，所提供内容均为地球化学工作者所必须了解的各种重要地质介质的地球化学基础数据。

本书供从事地球化学、岩石学、勘查地球化学、生态环境与农业地球化学、地质样品分析测试、矿产勘查、基础地质等领域的研究者阅读，也可供地球科学其它领域的研究者使用。

图书在版编目（CIP）数据应用地球化学元素丰度数据手册/迟清华，鄢明才编著. －北京：地质出版社，2007.12ISBN 978－7－116－05536－0Ⅰ. 应… Ⅱ. ①迟…②鄢…Ⅲ. 地球化学丰度－化学元素－数据－手册Ⅳ. P595－62中国版本图书馆CIP数据核字（2007）第185917号责任编辑：王永奉陈军中责任校对：李玫出版发行：地质出版社社址邮编：北京市海淀区学院路31号，100083电话：（010）82324508（邮购部）网址：电子邮箱：zbs@传真：（010）82310759印刷：北京地大彩印厂开本：889mm×1194mm 1/16印张：10.25字数：260千字印数：1－3000册版次：2007年12月北京第1版•第1次印刷定价：28.00元书号：ISBN 978－7－116－05536－0（如对本书有建议或意见，敬请致电本社；如本社有印装问题，本社负责调换）2关于应用地球化学元素丰度数据手册（代序）地球化学元素丰度数据，即地壳五个圈内多种元素在各种介质、各种尺度内含量的统计数据。

它是应用地球化学研究解决资源与环境问题上重要的资料。

将这些数据资料汇编在一起将使研究人员节省不少查找文献的劳动与时间。

这本小册子就是按照这样的想法编汇的。

Review of 'Detection, Estimation, and Modulation Theory, Part I'

612 case one is led to just the same equat,ions already given by Gibbs (even here, however, as was noted in the Brandeis lectures, but not in this book, the information theory viewpoint leads to a greater flexibility in our choice of the basic variables). The only place where new results are possible is in the extension to nonequilibrium problems, where previously no definite, unambiguous theory existed. However, the author’ s discussion of small deviations from equilibrium (transport coefficients, etc.) in Chapter 8, is conducted in a way that owes nothing at. all to the information theory approach. It reproduces only the results which had been found by Onsager, Casimir, Green, Kirkwood, Callen, Krrbo, Mori, and others prior to 1958, and which made no use of information theory. A more complete acco

Amari-Information geometry of divergence functions

∗ e-mail:
are explained intuitively. Most properties are already known, but here we give their new geometrical explanations. Bregman divergences are derived from convex functions. The Bregman divergence induces a dual structure through the Legendr´ e transformation. It gives a geometrical structure consisting of a Riemannian metric and dually ﬂat aﬃne connections, called the dually ﬂat Riemannian structure [1]. A dually ﬂat Riemannian manifold is a generalization of the Euclidean space, in which the generalized Pythagorean theorem and projection theorem hold. These two theorems provide powerful tools for solving problems in optimization, statistical inference and signal processing. We show that the Bregman type divergence is automatically induced from the dual ﬂatness of a Riemannian manifold. Then we study the class of invariant divergences [1, 11]. The invariance requirement comes from information monotonicity, which states that a divergence measure does not increase by coarse graining of information [12]. This leads to the class of f -divergences. The α-divergences are typical examples belonging to this class, which also includes the Kullback-Leibler divergence as a special case. This class of divergences induces an invariant Riemannian metric given by the Fisher information matrix and a pair of invariant dual aﬃne connections, the ±α-connections, which are not necessarily ﬂat. See [13] for more delicate problems occurring in the function space. When a family of unnormalized probability distributions, that is, a family of positive measures or arrays, is considered, we show that the α-divergence is the only class that is both invariant and dually ﬂat at the same time [14]. We further study the geometry derived from a general divergence in detail. This part is the original contribution of the present paper. A divergence endows a geometrical struc-

微相分离多组分聚合物体系

第六章微相分离多组分聚合物体系Scott扩展到聚合物共混体系均聚物共混时，混合的自由能变化为Flory-Huggins 的高分子溶液统计热力学理论溶剂A和高分子B混合时的自由能变化:v 为体积分数，n 为摩尔分数，χ1为HUggis 参数Φ为体积分数, V R 为摩尔链节体积，V 为总体积。

N：聚合度¾高分子体系中微相形态的演化非常缓慢，在样品制备过程中如果体系偶然陷落到某些非稳定状态，就比较难以转变到热力学稳定态¾实验观测与理论计算¾自洽场理论（self-consistent field theory, SCFT）：平均场理论，忽略热涨落¾决定嵌段共聚物微相分离的结构参数：嵌段共聚物微相分离时的嵌段混合焓正比于嵌段间的相互作用参数；微相分离的熵变与嵌段共聚物的聚合度（N）相关；嵌段共聚物组成也是影响相分离时的混合焓和混合熵的重要因素。

嵌段共聚物的组成（体积分数）f和组合参数反映了嵌段共聚物微相分离趋势的强弱对称嵌段共聚物¾自洽场理论的核心思想：对高分子链进行“粗粒化”（coarse-graining）处理¾把一个高分子看成是在空间中运动的“粒子”所走过的一条“路径”。

对含大量高分子的体系，把各个高分子之间的复杂的多体相互作用简化为一个共同的外加势场的作用。

¾1965年Edwards 自回避行走的高分子的线团尺寸（自回避行走的高分子在空间中的形状可以由一个在外场中进行扩散运动的粒子所走过的路径来表示，并且这个粒子的运动方程正好是薛定谔方程。

（高分子形态问题与量子力学之间的深刻联系）R∝N3/5g自洽场理论计算得到的两嵌段高分子本体的热力学相图（谱方法在Fourier空间严格求解）强分离限区域χN>100, S（立方体心相）、C（六方堆积柱分散相）和L（交替分层相）弱分离限区域CPS、S、C、G（双连续立方螺旋相）和L均相区χN<10.5M. W. Matsen, M. Schick. Phys. Rev. Lett., 1994, 72, 2660三嵌段（ABC ）聚合物f A 、f B 、NCore-shell sphere/cylinderSpheres in lamellae Rings on cylinderABC线性嵌段高分子在两维空间中的微相形态(a)层状相；(b)柱状相；(c)核-壳结构的柱状相；(d)四方相；(e)和(f)两种含球状相的层状相；(g)含球状相的柱状相Qiu F, et. al., Phys. Rev. E, 2004, 69, 1803动态密度泛函理论（自组装演化动态过程)稀溶液的组装由于嵌段弹性体中聚苯乙烯（PS ）内聚能密度较大，两端的PS 嵌段分别与其它链上的PS 嵌段聚集在一起，形成相畴为10~30nm 的球状物(称为微区)，作为物理交联点分散在聚丁二烯（PB)的连续相中。

光学冷加工-研磨加工基本知识

研磨加工基本知识讲义一、镜片加工流程及基本知识1、镜片加工流程：切削→研削→研磨→洗净2、切削的基本知识：切削：国内叫“粗磨”，国外叫NCG，为英文“球面创成”之缩写。

切削目的：去除材料硝材表面层，深度为0.5~0.6mm.。

由于硝材压型时精度不高，不加大加工余量就不能达到镜片所需尺寸（包括曲率、肉厚等）。

3、研削的基本知识：研削（也称精磨或砂挂），是镜片研磨前的极为重要的工序，研削加工的主要目的为：①加工出研磨工序所需要的表面精细度。

研削分为两道工序：A、第一道工序称S1，用1200#~1500#的钻石粒。

B、第二道工序称S2，用1500#~2000#的树指进行加工。

②加工出研磨工序所需要的球面精度。

③满足镜片中心肉厚要求，在规定的尺寸公差之内。

④研削品质的好坏对研磨后镜片的品质影响极大。

如研磨不良伤痕（キ）、砂目（ス）、肉厚、面不等不良均与研削有直接关系，研削品质的好坏决定研磨品质的优劣。

二、研磨加工基本知识：硝材在经过切削及研削，其基本尺寸及表面光洁度已经形成，但仍不能满足客户光学上的要求，必须进行研磨工序，研磨是获得光学表面的最主要的工序：1、研磨加工的目的：①去除精度的破坏层，达到规定的外观限度要求。

②精修面形，达到图面规置之不理的曲率半径R值，满足面本数NR要求及光圈局部允差（亚斯）的要求。

2、研磨的机理：①机械研削理论。

②化学学说。

③表面流动理论。

3、光圈的识别与度量（我们通常说的面即光圈）①什么是光圈？被检查镜片表面面形与标准曲率半径的原器面形有偏差时，它们之间含形成对称的契形空气间隙，从而形成等厚干涉条纹，有日光照射下可见到彩色光环（此时空气隙，呈环形对称），这种彩色的光环称为光圈，我们通常观察光圈数（即面本数）以红色光带为准。

这是因为红色光带较宽（波长范围为0.62um~0.78um），看起来清晰明亮。

②面本数的识别与度量有原器检查镜片时，如果二者是边缘接触（中间有空气层），从正方稍加压力P，干涉条纹从外向中心部移动即向内缩，称为低光圈或负光圈（图A），如果二者是从中间开始接触（边缘有空气隙），从正上方稍加压力P，干涉条纹从中心向边缘移动（或向外扩散）称为高光圈或正光圈（图B）③亚斯的识别与度量目前公司将面精度的中高、中低、垂边、分散或边等统称为亚斯，亚斯一定要满足作业标准的要求，超过标准含影响镜头的解像，所以亚斯是一个非常重要的指标，And grinding Basic knowledge handoutsLens processing processes and basic knowledge1, lens processing process:Wash cutting →grinding →grinding →2, the cutting of the basics:Cutting: Domestic called "coarse", abroad called the NCG, English spherical Creation "abbreviation.Cutting Objective: To remove the material the surface of the glass material layer and a depth of 0.5 to 0.6mm.Due to the type of glass material pressure accuracy is not high, do not increase the allowance can not be required to reach the lens size (including curvature, flesh, etc.).3, the grinding of the basics:Grinding (also known as grinding or sand hanging) is an extremely important step in front of the lens grinding Grinding main purpose:①machined surface fineness of the grinding step.Grinding is divided into two processes:A first process known as S1, 1200 # to 1500 # diamond particles.B, the second process known as S2, 1500 # ~ # 2000 resin processing.②processing spherical precision polishing step.(3) to meet the center of the lens flesh requirements within the specified dimensional tolerances.(4) grinding quality is good or bad quality of the lens grinding a great impact.Such as grinding bad scars (Cash), graining (su), flesh, ranging from bad to have a direct relationship with the grinding surface, the pros and cons of grinding quality determines the quality of the grinding quality.Grinding Basics:Nitrate material in its basic dimensions and surface finish has been formed after cutting and grinding, but it still can not meet the requirements of the customer optical polishing step mustbe carried out, is the most important step to obtain the optical surface polishing:1, the purpose of grinding:(1) removal of the accuracy of the destruction layer, to achieve the required appearance limit requirements.②the fine shave shaped to drawing requirements ignore the radius of curvature R, meet the requirements of the surface number NR requirements and aperture local tolerance (Elias).2, the grinding mechanism:①mechanical RESEARCH cut theory.(2) chemical theory.(3) surface flow theory.3, identification and measurement of the aperture (we usually say that the surface of the iris)①What is Aperture?Check the surface shape of the lens surface with the standard radius of curvature of the original surface shape deviation between them containing a symmetrical wedge-shaped air gap is formed, thereby forming fringes of equal thickness, the color can be seen under the sunlight halo (air gap annular symmetry), this halo of color called the iris aperture surface (the number), we usually observe the red band of light.This is because the red wide band of light (wavelength range 0.62um ~ 0.78um), looks clear and bright.②face identification and measurement of the number ofOriginal check lenses, if both the edge of the contact (the air layer in the middle), a little from the affirmative pressure P, interference fringes i.e. inwardly retracted portion moving from the center outward, as low the aperture or negative aperture (A) , if both are from the middle into contact with the air gap (edge), a little from the top of the positive pressure P, the interference fringes move from the center to the edge (or outward diffusion) called high aperture or positive aperture (Figure B)Determine the number of its surface is the red band of light as a standard vertical observation of several rings with that surface of the number of the Figure A Figure B is NR = -3, for NR = +3 this.(3) Elias identification and measurementSurface accuracy high, low, slouch, dispersed or side collectively referred to as Aspen, Aspen must meet the standard requirements of the job, more than the standard containing the impact of the resolution of the lens, so Aspen is a very important indicators。

Fusion 360 制图功能教程：绘制工程图纸说明书

Default Title Blocks ................................................................................................................ 9 Importing Custom Title Blocks ............................................................................................... 9 Drawing Templates................................................................................................................ 9
Your AU Expert(s)
Andrew de Leon is a senior principal user experience designer at Autodesk, Inc., with 20 years’ experience in the manufacturing industry and 11 years in user experience design. He has experience with AutoCAD software, AutoCAD Mechanical software, Inventor software, and Fusion பைடு நூலகம்60 software. He’s passionate about manufacturing and design, and enjoys solving difficult problems.

纹理物体缺陷的视觉检测算法研究--优秀毕业论文

摘要
在竞争激烈的工业自动化生产过程中，机器视觉对产品质量的把关起着举足轻重的作用，机器视觉在缺陷检测技术方面的应用也逐渐普遍起来。与常规的检测技术相比，自动化的视觉检测系统更加经济、快捷、高效与安全。纹理物体在工业生产中广泛存在，像用于半导体装配和封装底板和发光二极管，现代化电子系统中的印制电路板，以及纺织行业中的布匹和织物等都可认为是含有纹理特征的物体。本论文主要致力于纹理物体的缺陷检测技术研究，为纹理物体的自动化检测提供高效而可靠的检测算法。纹理是描述图像内容的重要特征，纹理分析也已经被成功的应用与纹理分割和纹理分类当中。本研究提出了一种基于纹理分析技术和参考比较方式的缺陷检测算法。这种算法能容忍物体变形引起的图像配准误差，对纹理的影响也具有鲁棒性。本算法旨在为检测出的缺陷区域提供丰富而重要的物理意义，如缺陷区域的大小、形状、亮度对比度及空间分布等。同时，在参考图像可行的情况下，本算法可用于同质纹理物体和非同质纹理物体的检测，对非纹理物体的检测也可取得不错的效果。在整个检测过程中，我们采用了可调控金字塔的纹理分析和重构技术。与传统的小波纹理分析技术不同，我们在小波域中加入处理物体变形和纹理影响的容忍度控制算法，来实现容忍物体变形和对纹理影响鲁棒的目的。最后可调控金字塔的重构保证了缺陷区域物理意义恢复的准确性。实验阶段，我们检测了一系列具有实际应用价值的图像。实验结果表明本文提出的纹理物体缺陷检测算法具有高效性和易于实现性。关键字: 缺陷检测；纹理；物体变形；可调控金字塔；重构
Keywords: defect detection, texture, object distortion, steerable pyramid, reconstruction
II

第五届统计物理与复杂系统会议日程(草稿)

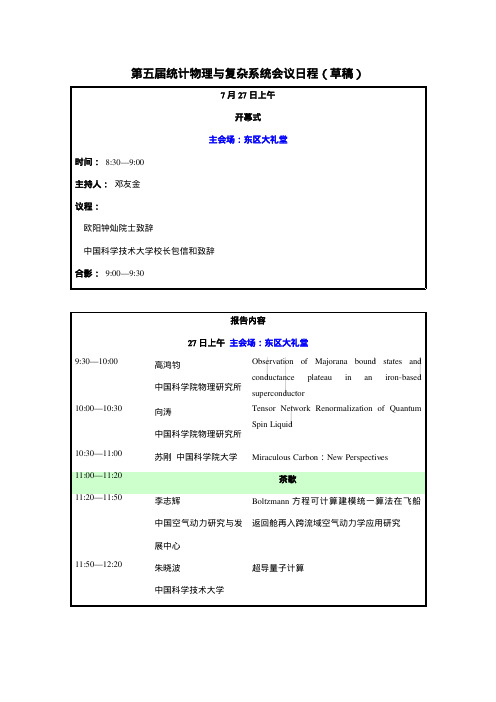
第五届统计物理与复杂系统会议日程（草稿）
7月27日上午
开幕式
主会场：东区大礼堂
时间：8:30—9:00
主持人：邓友金
议程：
欧阳钟灿院士致辞
中国科学技术大学校长包信和致辞
合影：9:00—9:30
主持人：
16:15—16:40郭文安北京师范大学Random-Singlet Phase in Disordered
Two-Dimensional Quantum Magnets
16:40—17:05陈锟
Rutgers University Feynman’s solution of the quintessential problem in solid state physics
17:05—17:25雷泽中国科学院理论
物理研究所
“Factor Field”下的“事件链”蒙特卡罗方法17:25—17:45
27日晚“统计物理与复杂系统研究的机遇与挑战”座谈会
20：00—21：30，丰大国际大酒店
11:50—12:20张潘中国科学院理论
物理研究所Solving Statistical Mechanics using Variational Autoregressive Networks
28日晚海报展示
19：30—21：30，丰大国际大酒店
闭幕式
主会场：东区大礼堂时间：17:20—17:50
主持人：陈晓松
议程：
优秀张贴报告奖颁奖
欧阳钟灿院士致闭幕辞
下一届会议举办单位介绍情况。

Finding community structure in networks using the eigenvectors of matrices

Finding community structure in networks using the eigenvectors of matrices
M. E. J. Newman
Department of Physics and Center for the Study of Complex Systems, University of Michigan, Ann Arbor, MI 48109–1040
We consider the problem of detecting communities or modules in networks, groups of vertices with a higher-than-average density of edges connecting them. Previous work indicates that a robust approach to this problem is the maximization of the beneﬁt function known as “modularity” over possible divisions of a network. Here we show that this maximization process can be written in terms of the eigenspectrum of a matrix we call the modularity matrix, which plays a role in community detection similar to that played by the graph Laplacian in graph partitioning calculations. This result leads us to a number of possible algorithms for detecting community structure, as well as several other results, including a spectral measure of bipartite structure in neteasure that identiﬁes those vertices that occupy central positions within the communities to which they belong. The algorithms and measures proposed are illustrated with applications to a variety of real-world complex networks.

卢柯 Revealing the Maximum Strength in Nanotwinned Copper

29.F.F.Balakirev et al.,Phys.Rev.Lett.102,017004(2009).30.F.Rullier-Albenque et al.,Phys.Rev.Lett.99,027003(2007).31.T.Senthil,Phys.Rev.B78,035103(2008).32.A.Kanigel et al.,Nat.Phys.2,447(2006).33.J.W.Loram,K.A.Mirza,J.R.Cooper,J.L.Tallon,J.Phys.Chem.Solids59,2091(1998).34.T.Yoshida et al.,J.Phys.Condens.Matter19,125209(2007).35.J.Zaanen,Nature430,512(2004).36.J.W.Loram,J.Luo,J.R.Cooper,W.Y.Liang,J.L.Tallon,J.Phys.Chem.Solids62,59(2001).37.C.Panagopoulos et al.,Phys.Rev.B67,220502(2003).38.H.J.A.Molegraaf,C.Presura,D.van der Marel,P.H.Kes,M.Li,Science295,2239(2002).39.S.Chakraborty,D.Galanakis,P.Phillips,/abs/0807.2854(2008).40.P.Phillips,C.Chamon,Phys.Rev.Lett.95,107002(2005).41.We acknowledge technical and scientific assistance fromS.L.Kearns,J.Levallois,and N.Mangkorntang andcollaborative support from H.H.Wen.This work wassupported by Engineering and Physical Sciences ResearchCouncil(UK),the Royal Society,Laboratoire National desChamps Magnétiques Pulsés,the French AgenceNationale de la Recherche IceNET,and EuroMagNET.Supporting Online Material/cgi/content/full/1165015/DC1Materials and MethodsFigs.S1and S2References22August2008;accepted21November2008Published online11December2008;10.1126/science.1165015Include this information when citing this paper.Revealing the Maximum Strengthin Nanotwinned CopperL.Lu,1*X.Chen,1X.Huang,2K.Lu1The strength of polycrystalline materials increases with decreasing grain size.Below a critical size,smaller grains might lead to softening,as suggested by atomistic simulations.The strongest size should arise at a transition in deformation mechanism from lattice dislocation activities to grain boundary–related processes.We investigated the maximum strength of nanotwinned copper samples with different twin thicknesses.We found that the strength increases with decreasing twin thickness,reaching a maximum at 15nanometers,followed by a softening at smaller values that is accompanied by enhanced strain hardening and tensile ductility.The strongest twin thickness originates from a transition in the yielding mechanism from the slip transfer across twin boundaries to the activity of preexisting easy dislocation sources.T he strength of polycrystalline materials increases with decreasing grain size,asdescribed by the well-known Hall-Petch relation(1,2).The strengthening originates from the fact that grain boundaries block the lattice dislocation motion,thereby making plastic defor-mation more difficult at smaller grain sizes.How-ever,below a certain critical size,the dominating deformation mechanism may change from lattice dislocation activities to other mechanisms such as grain boundary–related processes,and softening behavior(rather than strengthening)is expected (3,4).Such a softening phenomenon has been demonstrated by atomistic simulations,and a crit-ical grain size of maximum strength has been predicted(5–7).In pure metals,an impediment to determining the grain size that yields the highest strength is the practical difficulty of obtaining sta-ble nanostructures with extremely small structural domains(on the order of several nanometers).The driving force for growth of nanosized grains in pure metals,originating from the high excess en-ergy of numerous grain boundaries,becomes so large that grain growth may take place easily even at ambient temperature or below.Coherent twin boundaries(TBs),which aredefined in a face-centered cubic structure as the(111)mirror planes at which the normal stackingsequence of(111)planes is reversed,are known tobe as effective as conventional grain boundariesin strengthening materials.Strengthening has beenobtained in Cu when high densities of nanometer-thick twins are introduced into submicrometer-sized grains(8–10).In addition,coherent TBs aremuch more stable against migration(a fundamen-tal process of coarsening)than conventional grainboundaries,as the excess energy of coherent TBs isone order of magnitude lower than that of grainboundaries.Hence,nanotwinned structures areenergetically more stable than nanograined coun-terparts with the same chemical composition.Thestable nanotwinned structure may provide samplesfor exploring the softening behavior with very smalldomain sizes.Here,we prepared nanotwinned pureCu(nt-Cu)samples with average twin thicknessranging from a few nanometers to about100nm.High-purity(99.995%)Cu foil samples com-posed of nanoscale twin lamellae embedded insubmicrometer-sized grains were synthesized bymeans of pulsed electrodeposition.By increasingthe deposition rate to10nm/s,we succeeded inrefining the mean twin thickness(i.e.,the meanspacing between adjacent TBs,hereafter referredto as l)from a range of15to100nm down to arange of4to10nm(see supporting online ma-terial).The as-deposited Cu foils have an in-planedimension of20mm by10mm and a thicknessof30m m with a uniform microstructure.Shownin Fig.1,A to C,are transmission electron mi-croscopy(TEM)plane-view images of three as-deposited samples with l values of96nm,15nm,and4nm,respectively.The TEM images indicatethat some grains are irregular in shape,but low-magnification scanning electronic microscopyimages,both cross section and plane view,showthat the grains are roughly equiaxed in three di-mensions.Grain size measurements showed asimilar distribution and a similar average diam-eter of about400to600nm for all nt-Cu samples.Twins were formed in all grains(see the electrondiffraction pattern in Fig.1D),and observationsof twins in a large number of individual grainsrevealed no obvious change in the twin densityfrom grain to grain.Note that in all samples,theedge-on twins that formed in different grains arealigned randomly around the foil normal(growth)direction(8,11),in agreement with a strong[110]texture determined by x-ray diffraction(XRD).Foreach sample,twin thicknesses were measured froma large number of grains,which were detected fromnumerous TEM and high-resolution TEM(HRTEM)images,to generate a distribution.Figure1E illus-trates the492measurements for the sample withthe finest twins;the majority yielded spacings be-tween twins smaller than10nm,with a mean of4nm.For simplicity,each nt-Cu sample is iden-tified by its mean twin thickness;for example,thesample with l=4nm is referred to as nt-4.Figure2shows the uniaxial tensile true stress–true strain curves for nt-Cu samples of various lvalues.Also included are two stress-strain curvesobtained from a coarse-grained Cu(cg-Cu)andan ultrafine-grained Cu(ufg-Cu)that has a sim-ilar grain size to that of nt-Cu samples but is freeof twins within grains.Two distinct features areobserved with respect to the l dependence of themechanical behavior of nt-Cu.The first is the oc-currence of the l giving the highest strength.Allstress-strain curves of nt-Cu samples in Fig.2,Aand B,are above that of the ufg-Cu,indicating astrengthening by introducing twins into the sub-micrometer grains.However,such a strengthen-ing does not show a linear relationship with l.Forl>15nm(Fig.2A),the stress-strain curves shiftupward with decreasing l,similar to the strength-ening behavior reported previously in the nt-Cu(9,11)and nanocrystalline Cu(nc-Cu)(12–15)samples(Fig.3A).However,with further de-1Shenyang National Laboratory for Materials Science,Institute of Metal Research,Chinese Academy of Sciences,Shenyang 110016,P.R.China.2Center for Fundamental Research:Metal Structures in Four Dimensions,Materials Research Department, RisøNational Laboratory for Sustainable Energy,Technical Uni-versity of Denmark,DK-4000Roskilde,Denmark.*To whom correspondence should be addressed.E-mail: llu@ o n J a n u a r y 3 0 , 2 0 0 9 w w w . s c i e n c e m a g . o r g D o w n l o a d e d f r o mcreases of l down to extreme dimensions (i.e.,less than 10nm),the stress-strain curves shift down-ward (Fig.2B).As plotted in Fig.3A,the mea-sured yield strength s y (at 0.2%offset)shows a maximum value of 900MPa at l ≈15nm.The second feature is a substantial increase in tensile ductility and strain hardening when l <15nm.As seen in Fig.2,the tensile elongation of the nt-Cu samples increases monotonically with decreasing l .When l <15nm,the uniform ten-sile elongation exceeds that of the ufg-Cu sample,reaching a maximum value of 30%at the finest twin thickness.Strain-hardening coefficient (n )values were determined for each sample by fitting the uniform plastic deformation region to s =K 1+K 2e n ,where K 1represents the initial yield stress and K 2is the strengthening coefficient (i.e.,the strength increment due to strain hardening at strain e =1)(16,17).The n values determined for all the nt-Cu samples increase monotonically with de-creasing l (Fig.3B),similar to the trend of uniform elongation versus l .When l <15nm,n exceeds the value for cg-Cu (0.35)(16,17)and finally reaches a maximum of 0.66at l =4nm.The twin refinement –induced increase in n is opposite to the general observation in ultrafine-grained and nanocrystalline materials,where n continuously decreases with decreasing grain size (Fig.3B).The strength of the nt-Cu samples has been considered to be controlled predominantly by the nanoscale twins via the mechanism of slip trans-fer across the TBs (10,18),and it increases with decreasing l in a Hall-Petch –type relationship (9)similar to that of grain boundary strength-ening in nanocrystalline metals (12).Our re-sults show that such a relationship breaks down when l <15nm,although other structural pa-rameters such as grain size and texture are un-changed.The grain sizes of the nt-Cu samples are in the submicrometer regime,which is too large for grain boundary sliding to occur at room temperature,as expected for nanocrystalline ma-terials with grain sizes below 20nm (3).There-fore,the observed softening cannot be explained by the initiation of grain boundary –mediated mechanisms such as grain boundary sliding and grain rotation,as proposed by molecular dynam-ics (MD)simulations for nanocrystalline mate-rials (3).To explore the origin of the twin thickness giv-ing the highest strength,we carried out detailed structural characterization of the as-deposited sam-ples.HRTEM observations showed that in each sample TBs are coherent S 3interfaces associated with the presence of Shockley partial dislocations (as steps),as indicated in Fig.1D.These partial dislocations have their Burgers vector parallel to the twin plane and are an intrinsic structural fea-ture of twin growth during electrodeposition.The distribution of the preexisting partial dislocations is inhomogeneous,but their density per unit area of TBs is found to be rather constant among sam-ples with different twin densities.This suggests that the deposition parameters and the twin re-finement have a negligible effect on the nature ofTBs.Therefore,as a consequence of decreasing l ,the density of such TB-associated partial dislocations per unit volume increases.We also noticed that grain boundaries in the nt-Cu samples with l ≤15nm are characterized by straight segments (facets)that areoftenTrue strain (%)T r u e s t r e s s (M P a )True strain (%)Fig.2.Uniaxial tensile true stress –true strain curves for nt-Cu samples tested at a strain rate of 6×10−3s −1.(A )Curves for samples with mean twin thickness varying from 15to 96nm;(B )curves for samples with mean twin thickness varying from 4to 15nm.For comparison,curves for a twin-free ufg-Cu with a mean grain size of 500nm and for a cg-Cu with a mean grain size of 10m m areincluded.C200 nmA BbbDFig.1.TEM images of as-deposited Cu samples with 15nm.(C )l =4nm.(D )The same sample as (C)but at higher resolution,with a corresponding electron diffraction pattern (upper right inset)and a HRTEM image of the outlined area showing the presence of Shockley partials at the TB (lower right inset).(E )Distribution of the lamellar twin thicknesses determined from TEM and HRTEM images for l =4nm.REPORTSo n J a n u a r y 30, 2009w w w .s c i e n c e m a g .o r g D o w n l o a d e d f r o massociated with dislocation arrays (19),whereas in samples with coarser twins,grain boundaries are smoothly curved,similar to conventional grain boundaries.The microstrain measured by XRD was a negligible 0.01%for samples with l ≥15nm,but increased gradually from 0.038%to 0.057%when l decreased from 10to 4nm,which also indicates a gradual increase in the de-fect density.Recent experimental studies and MD simula-tions (3,20,21)have shown that an increase in the density of preexisting dislocations in nano-scale materials will cause softening.In the nt-Cu samples studied,both the dislocation arrays asso-ciated with the grain boundaries and the steps associated with the preexisting partial dislocations along TBs could be potential dislocation sources,which are expected to affect the initiation of plas-tic deformation (22)and to provide the disloca-tions required for the dislocation-TB interactions that cause work hardening.The preexisting par-tial dislocations can act as readily mobile dislo-cations,and their motion may contribute to the plastic yielding when an external stress is applied to the sample.The plastic strains induced by the motion of preexisting partial dislocations can be estimated as e =r 0b s d/M (where r 0is the initial dislocation density,b s is the Burgers vector of Shockley partial dislocation,d is the grain size,and M is the Taylor factor).Calculations showed that for the samples with l >15nm,the preexist-ing dislocations induce a negligibly small plastic strain (<0.05%).However,for the nt-4specimen,a remarkable amount of plastic strain,as high as 0.1to 0.2%,can be induced just by the motions of high-density preexisting dislocations at TBs (roughly 1014m −2),which could control the mac-roscopic yielding of the sample.The above anal-ysis suggests that for extremely small values of l ,a transition in the yielding mechanism can result in an unusual softening phenomenon in which the preexisting easy dislocation sources at TBs andgrain boundaries dominate the plastic deforma-tion instead of the slip transfer across TBs.Shockley partial dislocations are always in-volved in growth of twins during crystal growth,thermal annealing,or plastic deformation.Shock-ley partials might be left at TBs when the twin growth is interrupted.Therefore,the presence of Shockley partials at some TBs is a natural phe-nomenon.Although these preexisting dislocations may have a small effect on the mechanical be-havior of the samples with thick twins,the effect will be much more pronounced in the samples with nanoscale twins and/or with high preexist-ing TB dislocation densities such as those seen in deformation twins (23).To understand the extraordinary strain hard-ening,we analyzed the deformation structures of the tensile-deformed samples.In samples with coarse twins,tangles and networks of perfect dis-locations were observed within the lattice between the TBs (Fig.4A),and the dislocation density was estimated to be on the order of 1014to 1015m −2.In contrast,high densities of stacking faults and Shockley partials associated with the TBs were found to characterize the deformed structure of the nt-4sample (Fig.4,B and C),indicating the interactions between dislocations and TBs.Recent MD simulations (18,24,25)showed that when an extended dislocation (two Shockley partials connected by a stacking fault ribbon)is forced by an external stress into a coherent TB,it recom-bines or constricts into a perfect dislocation con-figuration at the coherent TB and then slips through the boundary by splitting into three Shockley par-tials.Two of them glide in the slip plane of the adjacent twin lamella,constituting a new extended dislocation,whereas the third one,a twinning par-tial,glides along the TB and forms a step.It is expected that with increasing strain,such an in-teraction process will generate a high density of partial dislocations (steps)along TBs and stack-ing faults that align with the slip planes in the twin lamellae,which may (or may not)connect to the TBs.Such a configuration of defects was observed,as shown in Fig.4C.The density of partial dislocations in the deformed nt-4sampleFig.3.Variation of (A )yield strength and (B )strain hardening coef-ficient n as a function of mean twin thickness for the nt-Cu samples.For comparison,the yield strength and n values fornc-Cu [▲(12),◀(13),▶(14),and ◆(15)],ufg-Cu[▾(9)],andcg-Cu samples reported in the literature are included.A maximum in the yieldstress is seen for thent-Cu with l =15nm,but this has not beenobserved for the nc-Cu,even when the grain size is as small as 10nm.0.00.20.40.60.8nor d (nm)0200400600800σy (M P a )λ or d (nm)020406080100120110100100010000λ2 nmTTB200 nmA CFig.4.(A )A typical bright TEM image of the deformed nt-96sample showing the tangling of lattice dislocations.(B )An HRTEM image of the nt-4sample tensile-deformed to a plastic strain of 30%,showing a high density of stacking faults (SF)at the TB.(C )The arrangement of Shockley partials and stacking faults at TBs within the lamellae in the nt-4sample.Triangles,Shockley partial dislocations associated with stacking faults;⊥,partials with their Burgers vector parallel to the TB plane.REPORTSo n J a n u a r y 30, 2009w w w .s c i e n c e m a g .o r g D o w n l o a d e d f r o mwas estimated to be 5×1016m −2on the basis of the spacing between the neighboring partials and l .This is two orders of magnitude higher than that of the preexisting dislocations and the lattice dislocations stored in the coarse twins.Such a finding suggests that decreasing the twin thick-ness facilitates the dislocation-TB interactions and affords more room for storage of dislocations,which sustain more pronounced strain hardening in the nt-Cu (26,27).These observations suggest that the strain-hardening behavior of nt-Cu samples is governed by two competing processes:dislocation-dislocation interaction hardening in coarse twins,and dislocation-TB interaction hardening in fine twins.With a refining of l ,the contribution from the latter mech-anism increases and eventually dominates the strain hardening,as revealed by the continuous increase of n values (Fig.3B).However,the former hard-ening mechanism usually leads to an inverse trend,diminishing with size refinement (17).Twins are not uncommon in nature,and they appear in various metals and alloys with different crystallographic structures.Extremely thin twin lamellae structures can possibly be achieved under proper conditions during crystal growth,plastic deformation,phase transformations,or thermal annealing of deformed structures.Our finding of the twin thickness giving maximum strength il-lustrates that the scale-dependent nature of plastic deformation of nanometer-scale materials is not necessarily related to grain boundary –mediated processes.This finding also provides insight into the development of advanced nanostructured materials.References and Notes1.E.O.Hall,Proc.Phys.Soc.London Ser.B 64,747(1951).2.N.J.Petch,J.Iron Steel Inst.174,25(1953).3.J.Schiøtz,K.W.Jacobsen,Science 301,1357(2003).4.S.Yip,Nature 391,532(1998).5.M.A.Meyers,A.Mishra,D.J.Benson,Prog.Mater.Sci.51,427(2006).6.P.G.Sanders,J.A.Eastman,J.R.Weertman,Acta Mater.45,4019(1997).7.C.C.Koch,K.M.Youssef,R.O.Scattergood,K.L.Murty,Adv.Eng.Mater.7,787(2005).8.L.Lu et al .,Acta Mater.53,2169(2005).9.Y.F.Shen,L.Lu,Q.H.Lu,Z.H.Jin,K.Lu,Scr.Mater.52,989(2005).10.X.Zhang et al .,Acta Mater.52,995(2004).11.L.Lu,Y.Shen,X.Chen,L.Qian,K.Lu,Science 304,422(2004);published online 18March 2004(10.1126/science.1092905).12.J.Chen,L.Lu,K.Lu,Scr.Mater.54,1913(2006).13.S.Cheng et al .,Acta Mater.53,1521(2005).14.Y.Champion et al .,Science 300,310(2003).15.Y.M.Wang et al .,Scr.Mater.48,1851(2003).16.A.Misra,X.Zhang,D.Hammon,R.G.Hoagland,Acta Mater.53,221(2005).17.M.A.Meyers,K.K.Chawla,in Mechanical Behavior of Materials ,M.Horton,Ed.(Prentice Hall,Upper Saddle River,NJ,1999),pp.112–135.18.Z.H.Jin et al .,Scr.Mater.54,1163(2006).19.X.H.Chen,L.Lu,K.Lu,J.Appl.Phys.102,083708(2007).20.X.Huang,N.Hansen,N.Tsuji,Science 312,249(2006).21.Z.W.Shan,R.K.Mishra,S.A.Syed Asif,O.L.Warren,A.M.Minor,Nat.Mater.7,115(2008).22.K.Konopka,J.Mizera,J.W.Wyrzykowski,J.Mater.Process.Technol.99,255(2000).23.Y.S.Li,N.R.Tao,K.Lu,Acta Mater.56,230(2008).24.S.I.Rao,P.M.Hazzledine,Philos.Mag.A 80,2011(2000).25.Z.H.Jin et al .,Acta Mater.56,1126(2008).26.M.Dao,L.Lu,Y.Shen,S.Suresh,Acta Mater.54,5421(2006).27.T.Zhu,J.Li,A.Samanta,H.G.Kim,S.Suresh,Proc.Natl.Acad.Sci.U.S.A.104,3031(2007).28.Supported by National Natural Science Foundation ofChina grants 50431010,50621091,50725103,and 50890171,Ministry of Science and Technology of China grant 2005CB623604,and the Danish National Research Foundation through the Center for FundamentalResearch:Metal Structures in Four Dimensions (X.H.).We thank N.Hansen,Z.Jin,W.Pantleon,and B.Ralph for stimulating discussions,X.Si and H.Ma for sample preparation,S.Zheng for TEM observations,and Y.Shen for conducting some of the tensile tests.Supporting Online Material/cgi/content/full/323/5914/607/DC1Materials and Methods Table S1References24October 2008;accepted 30December 200810.1126/science.1167641Control of Graphene ’s Properties by Reversible Hydrogenation:Evidence for GraphaneD.C.Elias,1*R.R.Nair,1*T.M.G.Mohiuddin,1S.V.Morozov,2P.Blake,3M.P.Halsall,1A.C.Ferrari,4D.W.Boukhvalov,5M.I.Katsnelson,5A.K.Geim,1,3K.S.Novoselov 1†Although graphite is known as one of the most chemically inert materials,we have found that graphene,a single atomic plane of graphite,can react with atomic hydrogen,which transforms this highly conductive zero-overlap semimetal into an insulator.Transmission electron microscopy reveals that the obtained graphene derivative (graphane)is crystalline and retains the hexagonal lattice,but its period becomes markedly shorter than that of graphene.The reaction with hydrogen is reversible,so that the original metallic state,the lattice spacing,and even the quantum Hall effect can be restored by annealing.Our work illustrates the concept of graphene as a robust atomic-scale scaffold on the basis of which new two-dimensional crystals with designed electronic and other properties can be created by attaching other atoms and molecules.Graphene,a flat monolayer of carbon atoms tightly packed into a honeycomb lattice,continues to attract immense interest,most-ly because of its unusual electronic properties and effects that arise from its truly atomic thick-ness (1).Chemical modification of graphene has been less explored,even though research on car-bon nanotubes suggests that graphene can be al-tered chemically without breaking its resilient C-C bonds.For example,graphene oxide is graphene densely covered with hydroxyl and other groups (2–6).Unfortunately,graphene oxide is strongly disordered,poorly conductive,and difficult to reduce to the original state (6).However,one can imagine atoms or molecules being attached to the atomic scaffold in a strictly periodic manner,which should result in a different electronic struc-ture and,essentially,a different crystalline mate-rial.Particularly elegant is the idea of attaching atomic hydrogen to each site of the graphene lattice to create graphane (7),which changes the hybridization of carbon atoms from sp 2into sp 3,thus removing the conducting p -bands and open-ing an energy gap (7,8).Previously,absorption of hydrogen on gra-phitic surfaces was investigated mostly in con-junction with hydrogen storage,with the research focused on physisorbed molecular hydrogen (9–11).More recently,atomic hydrogen chem-isorbed on carbon nanotubes has been studied theoretically (12)as well as by a variety of exper-imental techniques including infrared (13),ultra-violet (14,15),and x-ray (16)spectroscopy and scanning tunneling microscopy (17).We report the reversible hydrogenation of single-layer graphene and observed dramatic changes in its transport properties and in its electronic and atomic struc-ture,as evidenced by Raman spectroscopy and transmission electron microscopy (TEM).Graphene crystals were prepared by use of micromechanical cleavage (18)of graphite on top of an oxidized Si substrate (300nm SiO 2)and then identified by their optical contrast (1,18)and distinctive Raman signatures (19).Three types of samples were used:large (>20m m)crystals for Raman studies,the standard Hall bar de-vices 1m m in width (18),and free-standing mem-branes (20,21)for TEM.For details of sample fabrication,we refer to earlier work (18,20,21).1School of Physics and Astronomy,University of Manchester,M139PL,Manchester,UK.2Institute for Microelectronics Tech-nology,142432Chernogolovka,Russia.3Manchester Centre for Mesoscience and Nanotechnology,University of Manches-ter,M139PL,Manchester,UK.4Department of Engineering,Cambridge University,9JJ Thomson Avenue,Cambridge CB3OFA,UK.5Institute for Molecules and Materials,Radboud University Nijmegen,6525ED Nijmegen,Netherlands.*These authors contributed equally to this work.†To whom correspondence should be addressed.E-mail:Kostya@REPORTSo n J a n u a r y 30, 2009w w w .s c i e n c e m a g .o r g D o w n l o a d e d f r o m。

量子化学波谱计算基本流程

量子化学波谱计算基本流程英文回答:Quantum chemistry spectroscopy calculations involve several steps to determine the electronic structure and properties of molecules. The basic workflow typically includes the following steps:1. Geometry optimization: This step involves determining the most stable structure of the molecule by minimizing its potential energy. Various optimization algorithms, such as the gradient descent method, are used to find the equilibrium geometry.2. Basis set selection: A basis set is a set of mathematical functions used to approximate the wavefunction of the molecule. The choice of basis set affects the accuracy and computational cost of the calculations. Commonly used basis sets include the Gaussian basis set and the plane wave basis set.3. Electronic structure calculation: The electronic structure of the molecule is determined by solving theSchrödinger equation. This is typically done using methods such as Hartree-Fock theory, density functional theory (DFT), or post-Hartree-Fock methods like configuration interaction (CI) or coupled cluster (CC) theory. These methods provide information about the molecular orbitals, electronic energies, and properties.4. Spectroscopic property calculation: Once the electronic structure is determined, various spectroscopic properties can be calculated. For example, the vibrational frequencies can be obtained by solving the equations of motion for the nuclei using methods like harmonic or anharmonic vibrational analysis. The rotational spectrum can be calculated using methods like rigid rotor approximation or rotational-vibrational analysis.5. Comparison with experimental data: The calculated spectroscopic properties can be compared with experimental data to validate the accuracy of the calculations. Thishelps in understanding the molecular structure and dynamics, and also in predicting and interpreting experimental spectra.中文回答：量子化学波谱计算的基本流程包括以下几个步骤：1. 几何优化，通过最小化分子的势能来确定其最稳定的结构。

微米和纳米尺度内的复杂物质和流体

Sugarman & Prud’homme (1988) Chen et al.(2005)
Detection points at 25 cm and 200 cm
Parabolic Flow
Rf
avg. DNA velocity max.fluid velocity
Longer DNA higher velocity
Factors that affect DT: Colloid Particle size
Solved w/
Finite Element Method
z
For Different Channels
DNA Separation in Microcapillary
detector
T2 DNA after 100 s oscillatory Poiseuille flow
25 mm
l-DNA in microcapillary flow
Non-dilute DNA in Lattice Fluid Flow
Lattice Size = 40 X 20 X 40, corresponding to 20 x 10 x 20 mm3 box
Nc=50, 200, 400
We=100 Re=0.14
As the DNA concentration increases, the chain

v(r , r0 , f0 ) vs (r r0 ) vW (r , r0 ) Ω(r , r0 ) f0
Free space Wall correction
Force
Stokes Flow
0

p

耗散粒子动力学

保守力、耗散力、随机力
式中，分子团间的保守力为：
FijC
aij
(1
耗散力为： FijD
rijБайду номын сангаас)rˆij 0
D (rij
(rij 1)
(rij 1)
)(rˆij ij )rˆij
随机力为：
FijR R (rij )ij rˆij
式中,ωD与ωR为权重因子,为团间距离r的函数。当r>rC＝1时，ωD与ωR均为零。θij(t)为高斯分布的随机函数，即
FH理论适用于研究液体-液体、液体-固体的高分
子混合系统。依据FH理论,两成分的混合系统,其平
均自由能可表示为：
F kBT
A
NA
ln A
B
Nxij B
ln B AB
χ为A与B间的作用参数。即：
N A
ln[(1 A ) / A ] 1 2A
将FH理论与DPD方法比较,可推得参数α与χ间的
关系为：
体格子法的优点提出了DPD。 • 1995年Espanol和Warren提出DPD中耗散力和随机力中的权
函数必须满足涨落—耗散定理。 • Groot和Warren通过自由能将高分子系统的Flory-Huggins理
论与DPD方法相联系。 • Espanol、Warren 和Marsh 等人的工作奠定了耗散粒子动
Sc来改进系统性质，但是实施这种方法有很多困难。很显然最有效的增加 Sc的方法是增加rc，又考虑到对耗散力和随机力的计算要求是它们与rc3成比例。模拟复杂系统时计算耗时又是一个考虑的重要因素。在目前研究中，我们结
合修正的权函数和适当地增大截断半径来达到合理的计算
耗时。
2.4.2 保守力权函数的改进

球差电镜难熔高熵合金

球差电镜难熔高熵合金英文回答：The challenge of melting high-entropy alloys using a ball-milling technique is a topic that I have encountered in my research. High-entropy alloys are a class of materials that consist of multiple principal elements in roughly equal proportions. These alloys have garnered significant attention due to their unique mechanical and physical properties. However, their high melting points pose difficulties when it comes to processing them using conventional methods.One of the techniques commonly used to synthesize high-entropy alloys is ball milling, which involves the grinding of elemental powders in a high-energy ball mill. The mechanical energy generated during the milling process can lead to the formation of solid solutions or even amorphous phases, resulting in the desired high-entropy alloy. However, achieving a fully melted state is challengingbecause the high melting points of the constituent elements prevent complete melting.To overcome this challenge, researchers have explored various strategies. One approach is to add a liquid phase to the milling process, such as using a eutectic mixture or a reactive liquid. The presence of a liquid phase can lower the effective melting point of the alloy, facilitating the formation of a fully melted state. For example, in a study by Smith et al., they successfully melted a high-entropy alloy by adding a eutectic mixture of lithium chloride and potassium chloride to the milling process.Another strategy is to introduce a high-energy input to the system, such as using a high-power laser or anelectrical discharge. These energy sources can generate localized heating, allowing for the melting of the alloy in specific regions. By controlling the energy input, researchers can selectively melt the alloy without causing excessive damage to the milling equipment.In addition to the challenges of melting high-entropyalloys, there are also difficulties in characterizing their microstructures. The rapid solidification and high degree of disorder in these alloys make it challenging to obtain accurate structural information using conventional techniques. Advanced characterization methods, such as transmission electron microscopy and atom probe tomography, are often employed to study the microstructure and composition of high-entropy alloys.中文回答：球差电镜难以熔化高熵合金是我在研究中遇到的一个难题。

Differentiable coarse graining

Theoretical Computer Science 361(2006)111–129/locate/tcsDifferentiable coarse grainingJonathan E.Rowe a ,Michael D.V ose b ,∗,Alden H.Wright ca School of Computer Science,University of Birmingham,Englandb Computer Science Department,University of Tennessee,USAc Department of Computer Science,University of Montana,USAAbstractCoarse graining is deﬁned in terms of a commutative diagram.Necessary and sufﬁcient conditions are given in the continuously differentiable case.The theory is applied to linear coarse grainings arising from partitioning the population space of a simple Genetic Algorithm (GA).Cases considered include proportional selection,binary tournament selection,ranking selection,and mutation.A nonlinear coarse graining for ranking selection is also presented.A number of results concerning “form invariance”are given.Within the context of GAs,the primary contribution made is the illustration of a technique by which coarse grainings may be analyzed.It is applied to obtain a number of new coarse graining results.©2006Elsevier B.V .All rights reserved.Keywords:Differentiable;Coarse graining;Selection;Mutation;Form invariance1.IntroductionManaging complexity involves quotients (or some generalization thereof)if by “managing complexity”one intends to reduce complexity while simultaneously maintaining important aspects of ﬁdelity.The following diagram is an abstraction of the general scheme being considered.In that illustration,x ∈X represents state and h :X →X transforms plexity is managed by ,which maps state into a simpler form,and by ˜h which has reduced complexity by virtue of transforming simpliﬁed statex h −−−−→h(x) ⏐⏐ ⏐⏐x h−−−−→ h(x)(1)Maintaining important aspects of ﬁdelity is interpreted to mean the diagram commutes;both paths from x to h(x)yield identical results.Thus, can be regarded as deﬁning what aspects of ﬁdelity are maintained—if leeway exists in choosing it—or what aspects of ﬁdelity are capable of preservation—if there is virtually no leeway.If the diagram commutes,the reduced complexity model ˜h is the quotient of h corresponding to the coarse graining .The quotient ˜his referred to as a coarse graining of h (with respect to ),and h is said to be compatible with .∗Corresponding author.E-mail address:vose@ (M.D.V ose).0304-3975/$-see front matter ©2006Elsevier B.V .All rights reserved.doi:10.1016/j.tcs.2006.04.007112J.E.Rowe et al./Theoretical Computer Science361(2006)111–129Whereas modeling h in an approximate fashion(by relaxing commutativity of the diagram)is interesting,the central question of this paper is concerned with is whether one can do better than approximation,and if so,then how?Moreover, knowledge of what it is that can be exact may identify a useful starting point for what it is that later will be approximated or perturbed from.This abstract framework may provide a useful context in which to consider systems comprised of large collections of components interacting with each other(and with possibly some background environment).Assuming practical limitations to exact computation of the dynamics x,h(x),h◦h(x),...,approximation may be the best one can do.One would like to know,however,if that was the case or whether useful quotients did exist.It is natural to ask whether the underlying components could somehow be partitioned into a collection of disjoint subsets which could be considered as units in their own right.If obtaining a description of the dynamics of the subsets—in terms of the subsets alone—is possible,then the original system might be coarse grained into higher level units(the subsets)having dynamics compatible with the dynamics of the original system.This scenario will be made concrete by taking the system to be a Genetic Algorithm(GA).In that case the under-lying components comprise the search space,the environment is modeled by theﬁtness function(which determines competition between population members),and the state space is the set of possible populations.Whereas reading this paper should acquaint one with what it is primarily concerned with,a few remarks will be made—in the context of GAs—to help clarify what is not a primary concern.GA dynamics may equivalently be described with respect to various bases,typically either a string basis(elements of which correspond to particular strings)or a schema basis(elements of which correspond to particular schemata)[2]. Schemata are widely thought of as“coarse grained”,by virtue of being deﬁned in terms of collections of strings.1That notion,however,is to some extent arbitrary;one might likewise regard strings as“coarse grained”,by virtue of the fact that they are deﬁnable in terms of collections of schemata.In this paper,the coarse graining results concerning GAs do not coincide with a change of basis(as,for instance,moving from strings to schemata);in that case the quotient ˜h= ◦h◦ −1always exists,since a change of basis(i.e., )is invertible.2The primary contribution made by this paper is to introduce and illustrate a technique by which the possibility for coarse grainings may be analyzed.We are concerned with the application of analytical tools rather than establishing particular results about any speciﬁcﬁtness function.Rather than addressing the general situation,however,those tools speak to a special kind of coarse graining(differentiable coarse graining)which is introduced in Section3.The potential utility of those tools is demonstrated by obtaining a number of new coarse graining results.Roughly speaking,this paper is organized into four parts.Theﬁrst is this introduction and the next section where a few conceptual examples of general quotients are discussed.Second,a necessary and sufﬁcient condition characterizing quotients is described(assuming h is continuously differentiable,X is an open subset of aﬁnite-dimensional Euclidean space,etc.),followed by a reduction to special cases.Third,aspects of the theory of the Simple Genetic Algorithm [12]are reviewed in preparation for applying the necessary and sufﬁcient condition,and to relate coarse graining to the more general stochastic setting in which GAs are deﬁned.Fourth,the theory is applied to GAs in an investigation of quotients for selection and mutation,followed by a summary of results.2.Conceptual overviewA few examples are brieﬂy mentioned to make the general framework introduced above less abstract,to illustrate that in practice complex systems are frequently managed and understood with the aid of coarse grainings,and to provide some idea of where our applicationsﬁt within a more general context.It should be kept in mind that we must necessarily coarse grain some model of the real world,because the state space X and the transformation h are mathematical abstractions.1.Modeling the motion of a body by assuming it is rigid leads to a simple coarse graining(of that rigid model)where(x)is the center of gravity.Examples of this sort employ coarse grainings to transfer the domain of analysis to a simpliﬁed setting(namely,˜h acting on X).2.Invariants assert that the dynamics h(of some model of a physical system)is compatible with a coarse grainingunder which the quotient˜h is the identity map.For instance,E=mc2corresponds to the coarse graining (x)=1The term“coarse grained”is put in quotes to distinguish it from coarse graining as used in this paper.2In the invertible case, is called the conjugacy map between h and˜h,and they are said to be conjugate.J.E.Rowe et al./Theoretical Computer Science361(2006)111–129113E(x)−m(x)c2.Examples of this sort show the existence of coarse grainings may be used to constrain the analysis (in the original setting X)by invariants.3.The quantum mechanics describing the hardware of a computer is usually modeled by digital logic.A familiarcoarse graining(of that gate-level digital model)is the high-level gnu/linux interface seen by the C programmer.Examples of this sort suggest that the quotient˜h may be the primary object of concern;commutativity of the coarse graining( ◦h=˜h◦ )may serve as a proof of correctness for the implementation h.The quotient in the last example above is obtained only if the state transition x→h(x)corresponds to a number of microcycles which depends on x(namely,that number required for completion of the high-level service/command corresponding to x).This point is made to clarify the general phenomenon that even though a desirable quotient of a system’s single-step trajectoryx→h(x)→h2(x)→ (2)might not exist(think of h as being analogous to a single microcycle),it nevertheless could be the case that a multi-step trajectoryx→h p(x)(x)→h p(h p(x)(x))(x)→ (3)does admit useful quotients.The applications to genetic algorithms presented in Sections5–8,however,are limited to the single-step scenario(2)rather than the more general multi-step situation(3).Because models are coarse grained,an exact coarse graining(of a model)can be an approximation(to reality)if the model itself is an approximate one.This points to another reason why quotients may be signiﬁcant;they may aid in identifying tractable approximate models(i.e.,models which have useful quotients).If an“approximate coarse graining”of a model is desired(meaning that commutativity of diagram1is not strictly enforced),one might take that to be a strict coarse graining˜h of some h which approximates the model.In the situation where such h is not given,but a candidate and h: X→ X are known,a relevant observation is that a compatible h:X→X such that commutativity holds is trivial to construct from and h;3if the constructed h is deemed to approximate the intended model,then h could be regarded as an approximate coarse graining of the model.The applications to genetic algorithms presented in Sections5–8,however,are not concerned with approximation since the models being coarse grained are themselves exact.3.Differentiable coarse grainingThe following summarizes from[10].Rather than beginning with a coarse graining,one will be obtained as a byproduct of a continuously differentiable map.Constraining the framework for coarse graining in this way facilitates the application of differential calculus(most coarse grainings appearing in the Evolutionary Computation literature correspond to equivalence relations obtainable as a byproduct of linear—and thus trivially differentiable—maps).The hope is that this may provide a useful vantage point from which to consider coarse grainings,and,in some circumstances, to enable their computation.Let :V−→W be a continuously differentiable function between open subsets ofﬁnite-dimensional Euclidean spaces.4A path(with respect to )is a smooth function5 :[0,1]−→V such that ◦ is constant.The path is said to be from u to v provided (0)=u and (1)=v.Let the equivalence relation≡on V be deﬁned by u≡v⇐⇒there exists a path from u to vand let :V−→V/≡map element v to its equivalence class˜v.Equivalence classes are,in particular,path-connected components of level sets of .It follows that the image of any path is contained in some equivalence class.3Let h map elements of −1◦ (x)to elements of −1◦˜h◦ (x),where −1denotes inverse image(under ).4Such spaces sufﬁce for our purposes;a more general development is possible.5By smooth we mean a differentiable function whose differential(over the interior of the domain)has a continuous extension to the entire domain.114J.E.Rowe et al./Theoretical Computer Science 361(2006)111–129A continuously differentiable function h :V −→V is said to be compatible with ≡provided there exists a function ˜h for which the following diagram commutes:V h −−−−→V ⏐⏐ ⏐⏐ V /≡ h −−−−→V /≡(4)In that case ˜h is called the quotient of h (corresponding to the coarse graining );the quotient ˜h is referred to as a coarse graining of h (with respect to ),and h is said to be compatible with .Let T v be the tangent space of the equivalence class ˜v at v ,deﬁned byT v =L {d 0(1): is a path from v to w,for some w },where L {···}denotes the linear span of {···},and for any function f differentiable at x ,the differential of f at x is denoted by d f x .For any linear function L ,denote the kernel of L by K L .The proof of the following theorem can be found in [10].Theorem 1.A necessary and sufﬁcient condition for h to be compatible with ≡is that for all x ∈V ,d h x :T x −→K d h(x).Moreover ,T x is a subspace of K d x .The special case where is linear is referred to as linear coarse graining ,and the necessary and sufﬁcient condition reduces tod h x :K −→K .(5)If both h and are linear,then the situation reduces to the case considered in [9],h :K −→K .(6)It should be noted that the sort of coarse graining presented in this section,which we call differentiable coarse graining ,is not without loss of generality.The connected setC ={(x,y):y =sin (x −1),x =0}∪{(x,y):x =0}is a level set of the continuously differentiable function(x,y)=e −x −2(y −sin (x −1))but it cannot be an equivalence class (by our deﬁnition)since it is not path-connected [7].Our requirement that paths be smooth is also restrictive;the Koch snowﬂake curve is arc-connected,but nowhere differentiable [4],and,any closed set (e.g.,the Koch snowﬂake)can be a level set of a continuously differentiable function [3].As mentioned in the Introduction,commutativity of diagram 1is trivial when is invertible and ˜h = ◦h ◦ −1.More generally,if ˜h is a any coarse graining of h with respect to ,and if is invertible,then ◦˜h ◦ −1is a coarse graining of h with respect to ◦ .66Any map conjugate to a quotient is also a quotient,when quotient and coarse graining are general (i.e.,if the coarse graining is not required to be differentiable).J.E.Rowe et al./Theoretical Computer Science 361(2006)111–1291154.GAs and stochastic compatibilityThis section presents a brief summary of relevant background from [12]to introduce the mathematical framework in which Theorem 1will be applied.Let denote the stochastic transition function for a ﬁnite population GA 7over the search space ={0,...,n −1},8and let G be the corresponding inﬁnite population model.9The transition matrix Q of the GA’s Markov chain is deﬁned by the probability that (p)=q and satisﬁesQ p,q =r ! (G (p)j )rq j (rq j )!,(7)where r is the population size,and where the population represented by the n -dimensional real vector p contains rp j instances of j .The (completion of the)population representation space is the simplexn ={ x 0,...,x n −1 :x i 0,1T x =1},where ··· denotes a column vector,1is the vector of all 1s,10and ·T denotes transpose (of ·).Results of the previous section will be applied with h =G and V a path-connected (by smooth paths)neighborhood of n .Let ≡be an arbitrary equivalence relation over ,and let {0∗,...,(k −1)∗}be equivalence class representatives.The linear operator with k ×n matrix deﬁned byi,j =[i ∗≡j ](where [expression ]denotes 1if expression is true,and 0otherwise)lifts ≡to an equivalence relation between elements p,p ∈V byp ≡p ⇐⇒ p = p .(8)Note that1T =1T .(9)The set of equivalence classes (of ≡on V )isV /≡={V ∩( −1◦ v):v ∈V },where −1denotes inverse image.Therefore,V /≡is set-isomorphic to V (by )and the coarse graining (of the previous section)may without loss of generality be taken to coincide with the linear operator (of this section).This makes sense if is also chosen to coincide with ,since then the level sets (of )are precisely the elements of V /≡(they are path-connected by smooth paths since the inverse image of a point under a linear map is a subspace).The observations above may be summarized as follows.An arbitrary equivalence relation over gives rise to a linear operator ,which is naturally a linear coarse graining.Under that coarse graining,populations p and p are equivalent provided the populations they represent coincide when equivalent members of are regarded as indistinguishable (in view of the deﬁnition of ,that is what p = p asserts).Compatibility in the stochastic case generalizes the deﬁnition given in the previous section; is said to be compatible with ≡(also said to be compatible with )if and only ifp ≡p⇒∀q.Prob { (p)≡q }=Prob { (p )≡q }.(10)7 maps the current population to the next generation.8Whatever ﬁnite search space is intended,its elements may (in principle)be enumerated and referred to by integers.9G maps the current population to the expected next generation.10The dimension of the (column)vector 1is intentionally ambiguous,to be inferred from context.116J.E.Rowe et al./Theoretical Computer Science361(2006)111–129In that case,˜ deﬁned by˜ ( x)= (x)is referred to as the quotient of (with respect to ).It is known that the quotient˜ exists if and only if a quotient˜G of G exists(with respect to ),and the transition matrix˜Q for the Markov chain corresponding to˜ can be obtained from the formula for Q p,q(7)by replacing G by˜G,p by p,and q by q. Moreover,the image under of an evolutionary trajectory beginning from any population p and generated by the transition matrix Q is statistically indistinguishable from an evolutionary trajectory beginning from p and generated by˜Q[12].Therefore,the stochastic case has been reduced to a deterministic setting,and commutativity(of diagram4)is of particular interest—for the theory of GAs—when h coincides with G.Applications of linear coarse grainings in the following sections rely upon condition(5)to establish compatibility,where = and V is a neighborhood of n.A relevant observation is thereforeK =v:∀c∗.i≡c∗v i=0.(11)Whereas the discussion above relates the behavior of a GA(i.e., or equivalently Q)to that of its inﬁnite population model(G),that discussion is in the context of a linear coarse graining.Independent of that context,connections between their respective evolutionary trajectories are extensive;progress made with coarse graining G—by any means,whether the coarse graining is linear or not—reﬂects on (though in a less direct and more qualitative manner[12]).5.Proportional selection+mutationThe“proportional selection+mutation”case refers to the simple GA with proportionalﬁtness and mutation,but no crossover.The inﬁnite population model takes the formG(p)=Gp1T Gp,where G=MF is a n×n matrix and1is the vector of all1s.Here M is a column-stochastic11mutation matrix, where M i,j=Prob{j mutates to i},and F is a diagonalﬁtness matrix where F i,i=f i is theﬁtness of i(the vector f is referred to as theﬁtness function)[12].In particular,1T Gp=f T p.(12)The domain of immediate interest is n(the completion of the population representation space).Note that(12)implies 1T Gp does not vanish in a neighborhood of n,providedﬁtness is positive(i.e.,f has positive components).Positive ﬁtness will be assumed throughout the remainder of this paper.The following was established in[10]:Theorem2.Let be the stochastic transition function for a simple GA with(proportional)ﬁtness matrix F and mutation matrix M.Suppose positiveﬁtness and zero crossover,and let coarse graining correspond(as in Section4)to any equivalence relation≡over .Equivalent population members have identicalﬁtness if and only if F is compatible with .When F is compatible with ,a necessary and sufﬁcient condition for to be compatible with is that M is.If F is not compatible with ,then a necessary and sufﬁcient condition for to be compatible with is that the columns of M are equivalent.Theorem2speaks to the no-mutation case if M=I,and then the condition that columns of M be equivalent reduces to the requirement that≡has only one equivalence class(in ),all populations(in n)are equivalent,and then =1T. Theorem2is put into sharper focus by the following result(established in[11]),where e i refers to the i th column of the n×n identity matrix(indices begin with zero).11M is nonnegative,and1T M=1T.J.E.Rowe et al./Theoretical Computer Science 361(2006)111–129117Theorem 3.Let coarse graining correspond (as in Section 4)to any equivalence relation ≡over .A necessary and sufﬁcient condition for a mutation matrix M to be compatible with ≡is that for all i,j ,i ≡j ⇒Me i ≡Me j .Theorem 3provides a method by which a mutation operator can be constructed compatible with a given equivalence relation;whenever i ≡j ,choose columns i and j of M to differ by an element of K .Moreover,since K ⊂1⊥,obtaining column i by adding an element v ∈K to the j th column will not disturb the column stochasticity of M ,provided v +Me j is nonnegative.A mutation operator whose matrix M has equivalent columns (with respect to an equivalence relation ≡)is called restorative (with respect to ≡).This is equivalent to the existence of a vector w ∈ k (where k is the number of equivalence classes)such thatM =w 1T .Whereas is compatible with ≡for every ﬁtness function when mutation is restorative (by Theorems 2and 3),restorative mutation has a more remarkable property.Note that if x ∈ n ,then 1T x =1.Given restorative mutation,Mx =w 1T x =wis independent of x ∈ n .That observation implies the following:Theorem 4.Let mutation having corresponding matrix M be restorative with respect to ≡.For all u,v ∈ n ,Mu ≡Mv .A mutation operator is called universal when it is compatible with every equivalence relation over .Theorem 5.A mutation operator with matrix M is universal (i.e.,compatible with every equivalence relation ≡over )if and only if there exists w ∈ n and 0 such thatM =(1− )I + w 1Tis nonnegative .Proof.Suppose that the displayed matrix above is nonnegative.Since1T M =(1− )1T + 1T =1Tthe matrix M is both nonnegative and column stochastic,and therefore corresponds to a mutation operator.Let i ≡j ,so that e i −e j ∈K (where the coarse graining corresponds to an equivalence relation ≡over ).Note thatMe i =(1− )e i + w.HenceMe i −Me j =(1− )(e i −e j )∈K .Conversely,suppose M is universal.First,consider the special case n =2(the case n =1is trivial).M = a b c d = a −b 00d −c + b c11 ,where a +c =1=b +d .Therefore a −b =d −c .If b =c =0then M =I ,so let =0and choose w arbitrarily.Otherwise,let =b +c and choose w = −1 b,c to obtainM = I + w 1T .Since 1T =1T M =( + )1T ,it follows that =1− .118J.E.Rowe et al./Theoretical Computer Science361(2006)111–129Next,consider the general case n>2.Let0∗≡h and k=h⇒1∗≡k.Let i=j both be equivalent to1∗.Since M is compatible(with≡),Me i= Me j.Multiplying through by e T1leads tok≡1∗M k,i=k≡1∗M k,j.Since M is column stochastic(and k=h⇔1∗≡k),the above is equivalent to1−M h,i=1−M h,j.It follows that with the possible exception of the diagonal elements,M has identical rows(h,i,j are arbitrary,subject to being distinct)and can therefore be expressed asM=D+ w1T,where D is diagonal,w∈ n,and0 .Multiplying through by1T yields1T=1T D+ 1T.It follows that D=(1− )I.Unlike universal mutation,restorative mutation cannot(for general≡)be arbitrarily close to the identity,since otherwiselimM→IMe j= e jis independent of j,and consequently[i∗≡j]is independent of j(i.e.,there can be only one equivalence class). Hence,when≡has more than one class and if there exist equivalent population members with unequalﬁtness,it is impossible for to remain compatible(in the proportional selection+mutation case)as mutation vanishes.5.1.Form invarianceIf h is compatible with a coarse graining corresponding(as in Section4)to any equivalence relation≡over ,the corresponding quotient is given by˜h= ◦h◦D T,where D is the diagonal matrixD i,i=1/ j≡i1.Moreover, D T=I,which implies x≡D T x,and therefore when h is compatible,h(x)≡h◦D T x and so h(x)= ◦h◦D T x(see[12]).To say h is form invariant under coarse graining is to assert that˜h has the same form as h(when h is compatible with≡).Theorem6.Proportional selection+mutation is form invariant.J.E.Rowe et al./Theoretical Computer Science 361(2006)111–129119Proof.Theorems 2and 3imply M is compatible when G is,and therefore◦G ◦D Tx =( M)FD T x 1T ( M)FD T x =( MD T )FD T x 1T ( MD T )FD T x=( MD T )( FD T )x 1T ( MD T )( FD T )x=M F x 1T MF x .Note that1T M=1T MD T =1T MD T =1T D T =1T D T =1T I =1T hence M is column stochastic (i.e.,a mutation matrix).Moreover, F is a diagonal ﬁtness matrix, F i,j = a,b,c i,a F a,b D b,c j,c = a,b,c [a ≡i ∗][a =b ]f a [b =c ] ≡c 1[c ≡j ∗]=[i ∗≡j ∗]c ≡i ∗f c ≡c 1.6.Binary tournament selection +mutationA zero mutation,zero crossover,tournament selection GA with tournament size t and ﬁtness function f has corre-sponding inﬁnite population model [12]F (p)i =t ! v ∈X t n[f j f i ](v/t)j [f j <f i ](v/t)j (y)d y j<n p v j j v j !,whereX t n ={ x 0,...x n −1 :x i ∈Z 0,1T x =t }and is any continuous increasing probability density over [0,1].Binary tournament selection refers to the result of choosing t =2and taking the limit as tends to point mass at 1.Assuming injective ﬁtness (which will be assumed for the remainder of this paper),the result isF (p)i =p 2i +2p i jp j [f j <f i ].It follows that(d F x v)i =2v i x i +2l [f l <f i ](v i x l +x i v l ).(13)Note that (13)is a symmetric expression in x and v ,and therefore d F x v =d F v x is linear in both x and v .In view of this,the compatibility condition is that for all x ∈V ,and for all v ∈K ,d F x v = hx h d F e h v ∈K .120J.E.Rowe et al./Theoretical Computer Science361(2006)111–129Since K is a subspace,compatibility is therefore equivalent to the condition that for all h,v∈K ⇒d F e h v∈K .(14) Moreover,the i th component of the differential above simpliﬁes(from(13))to(d F eh v)i=2v i[f h<f i]+2[h=i]l[f l f i]v l.(15)Let be a permutation of{0,...,n−1}such that i<j⇐⇒f (i)<f (j)and let≡be any equivalence relation on for which the equivalence classes areﬁtness-contiguous,meaning they are{ (0),..., (z0)},{ (z0+1),..., (z1)},...,{ (z k−2+1),..., (z k−1)}for some−1=z−1<z0<···<z k−1=n−1.Let the equivalence class representative of the c th class be c∗= (z c). It follows that if b<c then everything equivalent to b∗hasﬁtness less than everything equivalent to c∗.An equivalence relation is referred to asﬁtness-contiguous when its equivalence classes are.The following was established in[10]: Theorem7.Binary tournament selection is compatible with≡if and only if the equivalence relation isﬁtness-contiguous.Incorporating mutation complicates matters.Let mutation have corresponding matrix M,and consider the GA as above with mutation included(i.e.,“binary tournament selection+mutation”);its inﬁnite population model is G=M F with differential d G x=M d F x.Choosing h= (0)in(15)yieldsd G e(0)=M d F e(0)=2M.Therefore,a necessary condition for G to be compatible with≡is that M is.Just as d F x v=d F v x is linear in both x and v,the same is true of d G x,and consequently(as for(14))compatibility of G is equivalent(via(11)and(15))to the condition that for all h and c∗,v∈K ⇒0=jv j[f h<f j]i≡c∗M i,j+l[f l f h]v li≡c∗M i,h.(16)Sinceﬁtness is injective,and v∈K implies1T v=0,this can be rewritten asv∈K ⇒0=jv j[f h<f j]i≡c∗(M i,j−M i,h).(17)This condition is linear in v,and so attention may be restricted to a basis for K ,which(by(11))can be taken to beB=r∗v:v i=0,v i=0⇒i≡r∗.The extent of representative r∗(with respect to f and≡)is deﬁned asE r∗=i:mink≡r∗f k f i maxk≡r∗f k.A mutation operator with matrix M is called contiguous(with respect to f and≡)if it is compatible with≡and for all r∗,i,j∈E r∗ ⇒Me i≡Me j.Theorem8.Let be the stochastic transition function for a simple GA withﬁtness f,binary tournament selection F,and mutation matrix M.Suppose injectiveﬁtness and zero crossover,and let coarse graining correspond。