SAT物理考试题(2套)
SAT2物理习题 Electric Potential and Capacitance

SAT2物理习题Electric Potential and Capacitance以下是小编为大家整理的SAT2物理习题Electric Potential and Capacitance,一定会大家sat备考有帮助的,赶快下载吧。
SAT2物理习题Electric Potential and Capacitance1. Which of the following statements is/are true?I. If the electric field at a certain point is zero, then the electric potential at the same point is also zero.II. If the electric potential at a certain point is zero, then the electric field at the same point is also zero.III. The electric potential is inversely proportional to the strength of the electric field.(A) I only(B) II only(C) I and II only(D) I and III only(E) None are true2. If the electric field does negative work on a negative charge as the charge undergoes a displacement from position A to position B within an electric field, then the electrical potential energy(A) is negative(B) is positive(C) increases(D) decreases(E) Cannot be determined from the information given3. The work required to assemble the system shown above, bringing each charge in from an infinite distance, is equal to4. Negative charges are accelerated by electric fields toward points(A) at lower electric potential(B) at higher electric potential(C) where the electric field is zero(D) where the electric field is weaker(E) where the electric field is stronger5. A charge q experiences a displacement within an electric field from position A to position B. The change in the electrical potential energy is △UE, and the work done by the electric field during this displacement is WE, Then6. Which points in the uniform electric field (between the plates of the capacitor) shown above lie on the same equipotential?(A) 1 and 2 only(B) 1 and 3 only(C) 2 and 4 only(D) 3 and 4 only(E) 1,2,3, and 4 all lie on the same equipotential, since the electric field is uniform.7. The potential at point A in an electric field is 10V higher than at point B. If a negative charge, q=-2 C, is moved from point A to point B, then the potential energy of this charge will(A) decrease by 20 J(B) decrease by 5 J(C) increase by 5 J(D) increase by 20 J(E) increase by 100 J8. A parallel-plate capacitor is charged to a potential difference of △V; this results in a charge of +Q on one plate and a charge of -Q on the other. The capacitor is disconnected from the charging source, and a dielectric is then inserted. What happens to the potential difference and the stored electrical potential energy?(A) The potential difference decreases, and the stored electrical potential energy decreases.(B) The potential difference decreases, and the stored electrical potential energy increases.(C) The potential difference increases, and the stored electrical potential energy decreases.(D) The potential difference increases, and the stored electrical potential energy increases.(E) The potential difference decreases, and the stored electrical potential energy remains unchanged.9. How much work would the electric field (created by the stationary charge Q) perform as a charge q is moved from point A to B along the curved path shown?(VA = 200 V, VB = 100 V,q = -0.05 C, length of line segment AB = 10 cm, length of curved path = 20 cm.)(A) -10 J(B) -5 J(C) +5 J(D) +10 J(E) +20 J。
上海美国国际中学高三物理联考试卷含解析

上海美国国际中学高三物理联考试卷含解析一、选择题:本题共5小题,每小题3分,共计15分.每小题只有一个选项符合题意1. 假设地球同步卫星的轨道半径是地球半径的n倍,则A.同步卫星运行速度是第一宇宙速度的倍,B.同步卫星的运行速度是第一宇宙速的倍C.同步卫星的运行速度是地球赤道上物体随地球自转速度的倍D.同步卫星的向心加速度是地球表面重力加速度的倍参考答案:B2. (单选)A、B为电荷量分别为+Q和﹣Q的两个等量异种点电荷.c、d为A、B连线上的两点,且Ac=Bd,如图所示.关于c、d两点间电场强度的情况是()匀速圆周运动,磁场方向竖直向下,其俯视图如图所示.若小球运动到A点时,绳子忽然断开.关于小球在绳断开后可能的运动情况,下列说法中正确的是A.小球仍作逆时针方向的匀速圆周运动,半径不变B.小球仍作逆时针方向的匀速圆周运动,但半径减小C.小球将作顺时针方向的匀速圆周运动,半径不变D.小球将作顺时针方向的匀速圆周运动,但半径增大参考答案:ACD4. 下列关于物体运动的情况,可能存在的是()A.物体具有加速度,而速度为零 B.物体的加速度为零,而其速度很大C.加速度逐渐减小,而速度逐渐增大 D.加速度逐渐变大,而速度保持不变参考答案:答案:ABC5. 力学中,选定下列哪组物理量为国际单位制中的基本物理量[LU1]:()A.力、长度、质量. B.位移、质量、时间.C.长度、力、时间. D.长度、质量、时间.参考答案:二、填空题:本题共8小题,每小题2分,共计16分6. 图1为验证牛顿第二定律的实验装置示意图。
图中打点计时器的电源为50Hz的交流电源,打点的时间间隔用Δt表示。
在小车质量未知的情况下,某同学设计了一种方法用来研究“在外力一定的条件下,物体的加速度与其质量间的关系”。
(1)完成下列实验步骤中的填空:①平衡小车所受的阻力:小吊盘中不放物块,调整木板右端的高度,用手轻拨小车,直到打点计时器打出一系列________的点。
SAT物理考试题(2套)

SAT物理考试-Inclined Planeswe know that when people are placed on slippery inclines, they slide down the slope. We also know that slides can sometimes be sticky, so that when you are at the top of the incline, you need to give yourself a push to overcome the force of static friction. As you descend a sticky slide, the force of kinetic friction opposes your motion. In this section, we will consider problems involving inclined planes both with and without friction. Since they’re simpler, we’ll begin with frictionless planes.Suppose you place a 10 kg box on a frictionless 30º inclined plane and release your hold, allowing the box to slide to the ground, a horizontal distance of d meters and avertical distance of h meters.Before we continue, let’s follow those three important preliminary steps for solving problems in mechanics:1. Ask yourself how the system will move: Because this is a frictionless plane,there is nothing to stop the box from sliding down to the bottom. Experience suggests that the steeper the incline, the faster an object will slide, so we can expect the acceleration and velocity of the box to be affected by the angle of the plane.2. Choose a coordinate system: Because we’re interested in how the box slidesalong the inclined plane, we would do better to orient our coordinate system to the slope of the plane. The x-axis runs parallel to the plane, where downhill is the positive xdirection, and the y-axis runs perpendicular to the plane, where up is the positive ydirection.3. Draw free-body diagrams: The two forces acting on the box are the force ofgravity, acting straight downward, and the normal force, acting perpendicular to theinclined plane, along the y-axis. Because we’ve oriented our coordinate system to the slope of the plane, we’ll have to resolve the vector for the gravitational force, mg, into its x- and y-components. If you recall what we learned about vector decomposition inChapter 1, you’ll know you can break mg down into a vector of magnitude cos 30º in the negative y direction and a vector of magnitude sin 30º in the positive x direction. The result is a free-body diagram that looks something like this:Decomposing the mg vector gives a total of three force vectors at work in this diagram: the y -component of the gravitational force and the normal force, which cancel out; and the x -component of the gravitational force, which pulls the box down the slope. Note that the steeper the slope, the greater the force pulling the box down the slope.Now let’s solve some problems. For the purposes of these problems, take the acceleration due to gravity to be g = 10 m/s 2. Like SAT II Physics, we will give you the values of the relevant trigonometric functions: cos 30 = sin 60 = 0.866, cos 60 = sin 30 = 0.500.1. What is the magnitude of the normal force?2. What is the acceleration of the box?3. What is the velocity of the box when it reaches the bottom of the slope?4.What is the work done on the box by the force of gravity in bringing it to the bottom of the plane?1. What is the magnitude of the normal force?The box is not moving in the y direction, so the normal force must be equal to the y -component of the gravitational force. Calculating the normal force is then just a matter of plugging a few numbers in for variables in order to find the y -component of the gravitational force:2. ?We know that the force pulling the box in the positive x direction has a magnitude of mg sin 30. Using Newton’s Second Law, F = ma , we just need to solve for a :3. What is the velocity of the box when it reaches the bottom of the slope?Because we’re dealing with a frictionless plane, the system is closed and we can invoke the law of conservation of mechanical energy. At the top of the inclined plane, thebox will not be moving and so it will have an initial kinetic energy of zero ().At the bottom of the slope, all the box’s potential energy will have been converted into kinetic energy. In other words, the kinetic energy, 1⁄2 mv2, of the box at the bottom of the slope is equal to the potential energy, mgh, of the box at the top of the slope. Solving for v, we get:4. What is the work done on the box by the force of gravity in bringing it to the bottom of the inclined plane?The fastest way to solve this problem is to appeal to the work-energy theorem, which tells us that the work done on an object is equal to its change in kinetic energy. At the top of the slope the box has no kinetic energy, and at the bottom of the slope its kinetic energy is equal to its potential energy at the top of the slope, mgh. So the work done on the box is:Note that the work done is independent of how steep the inclined plane is, and is only dependent on the object’s change in height when it slides down the plane.Frictionless Inclined Planes withLet’s bring together what we’ve learned about frictionless inclined planes and pulleys on tables into one exciting über-problem:Assume for this problem that —, wNow let’s tackle a couple of questions:1. What is the acceleration of the masses?2. What is the velocity of mass m after mass M has fallen a distance h?1. What is the acceleration of the masses?First, let’s determine the net force acting on each of the masses. Applying Newton’s Second Law we get:Adding these two equations together, we find that . SBecause , th. S. T. BFinally, note that the velocity of mass m is in the uphill direction.As with the complex equations we encountered with pulley systems above, you needn’t trouble yourself with memorizing a formula like this. If you understand the principles at work in this problem and would feel somewhat comfortable deriving this formula, you know more than SAT II Physics will likely ask of you.Inclined Planes With FrictionThere are two significant differences between frictionless inclined plane problems and inclined plane problems where friction is a factor:1. There’s an extra force to deal with. The force of friction will oppose the downhillcomponent of the gravitational force.2. We can no longer rely on the law of conservation of mechanical energy.Because energy is being lost through the friction between the mass and the inclined plane, we are no longer dealing with a closed system. Mechanical energy is not conserved.Consider the 10 kg box we encountered in our example of a frictionless inclined plane.This time, though, the inclined plane has a coefficient of kinetic friction of . HNow let’s ask some questions about the motion of the box.1. What is the force of kinetic friction acting on the box?2. What is the acceleration of the box?3. What is the work done on the box by the force of kinetic friction?What is the force of kinetic friction acting on the box?The normal force acting on the box is 86.6 N, exactly the same as for the frictionless inclined plane. The force of kinetic friction is defined as , sand N:Remember, though, that the force of friction is exerted in the negative x direction, so the correct answer is –43.3 N.What is the acceleration of the box?The net force acting on the box is the difference between the downhillgravitational force and the force of friction: . UBecause , th. RNote that the amount of work done is negative, because the force of friction acts in the opposite direction of the displacement of the box.we know that when people are placed on slippery inclines, they slide down the slope. We also know that slides can sometimes be sticky, so that when you are at the top of the incline, you need to give yourself a push to overcome the force of static friction. As you descend a sticky slide, the force of kinetic friction opposes your motion. In this section, we will consider problems involving inclined planes both with and without friction. Since they’re simpler, we’ll begin with frictionless planes.Suppose you place a 10 kg box on a frictionless 30º inclined plane and release your hold, allowing the box to slide to the ground, a horizontal distance of d meters and a vertical distance of h meters.Before we continue, let’s follow those three important preliminary steps for solving problems in mechanics:1. Ask yourself how the system will move: Because this is a frictionless plane,there is nothing to stop the box from sliding down to the bottom. Experience suggests that the steeper the incline, the faster an object will slide, so we can expect the acceleration and velocity of the box to be affected by the angle of the plane.2. Choose a coordinate system: Because we’re interested in how the box slidesalong the inclined plane, we would do better to orient our coordinate system to the slope of the plane. The x-axis runs parallel to the plane, where downhill is the positive xdirection, and the y-axis runs perpendicular to the plane, where up is the positive ydirection.3. Draw free-body diagrams: The two forces acting on the box are the force ofgravity, acting straight downward, and the normal force, acting perpendicular to theinclined plane, along the y-axis. Because we’ve oriented our coordinate system to the slope of the plane, we’ll have to resolve the vector for the gravitational force, mg, into its x- and y-components. If you recall what we learned about vector decomposition inChapter 1, you’ll know you can break mg down into a vector of magnitude cos 30º in the negative y direction and a vector of magnitude sin 30º in the positive x direction. The result is a free-body diagram that looks something like this:Decomposing the mg vector gives a total of three force vectors at work in this diagram: the y-component of the gravitational force and the normal force, which cancel out; and the x-component of the gravitational force, which pulls the box down the slope.Note that the steeper the slope, the greater the force pulling the box down the slope.Now let’s solve some problems. For the purposes of these problems, take the acceleration due to gravity to be g = 10 m/s2. Like SAT II Physics, we will give you the values of the relevant trigonometric functions: cos 30 = sin 60 = 0.866, cos 60 = sin 30 =0.500.SAT物理考试-SpringsThe that springs exhibit applies equally to objects moving in a circular path and to the various wave phenomena that we’ll study later in this book. So before we dig in to the nitty-gritty of your typical SAT II Physics spring questions, let’s look at some generalfeatures of harmonic motion.and Harmonic MotionConsider the following physical phenomena:•When you drop a rock into a still pond, the rock makes a big splash, which causes ripples to spread out to the edges of the pond.•When you pluck a guitar string, the string vibrates back and forth.•When you rock a small boat, it wobbles to and fro in the water before coming to rest again.•When you stretch out a spring and release it, the spring goes back and forth between being compressed and being stretched out.There are just a few examples of the widespread phenomenon of oscillation.Oscillation is the natural world’s way of returning a system to its equilibrium position, the stable position of the system where the net force acting on it is zero. If you throw a systemoff-balance, it doesn’t simply return to the way it was; it oscillates back and forth about the equilibrium position.A system oscillates as a way of giving off energy. A system that is thrown off-kilter has more energy than a system in its equilibrium position. To take the simple example of a spring, a stretched-out spring will start to move as soon as you let go of it: that motion is evidence of kinetic energy that the spring lacks in its equilibrium position. Because of the law of conservation of energy, a stretched-out spring cannot simply return to its equilibrium position; it must release some energy in order to do so. Usually, this energy is released as thermal energy caused by friction, but there are plenty of interesting exceptions. For instance, a plucked guitar string releases sound energy: the music we hear is the result of the string returning to its equilibrium position.The movement of an oscillating body is called harmonic motion. If you were to graph the position, velocity, or acceleration of an oscillating body against time, the result would be a sinusoidal wave; that is, some variation of a y = a sin bx or a y = a cos bx graph. This generalized form of harmonic motion applies not only to springs and guitar strings, but to anything that moves in a cycle. Imagine placing a pebble on the edge of a turntable, and watching the turntable rotate while looking at it from the side. You will see the pebble moving back and forth in one dimension. The pebble will appear to oscillate just like a spring: it will appear to move fastest at the middle of its trajectory and slow to a halt and reverse direction as it reaches the edge of its trajectory.This example serves two purposes. First, it shows you that the oscillation of springs is just one of a wide range of phenomena exhibiting harmonic motion. Anything that moves in a cyclic pattern exhibits harmonic motion. This includes the light and sound waves without which we would have a lot of trouble moving about in the world. Second, we bring it up because SAT II Physics has been known to test students on the nature of the horizontal or vertical component of the motion of an object in circular motion. As you can see, circular motion viewed in one dimension is harmonic motion.Though harmonic motion is one of the most widespread and important of physical phenomena, your understanding of it will not be taxed to any great extent on SAT II Physics. In fact, beyond the motion of springs and pendulums, everything you will need toknow will be covered in this book in the chapter on Waves. The above discussion is mostly meant to fit your understanding of the oscillation of springs into a wider context.The Oscillation of a SpringNow let’s focus on the harmonic motion exhibited by a spring. To start with, we’ll imagine a mass, m, placed on a frictionless surface, and attached to a wall by a spring. In its equilibrium position, where no forces act upon it, the mass is at rest. Let’s label this equilibrium position x = 0. Intuitively, you know that if you compress or stretch out the spring it will begin to oscillate.Suppose you push the mass toward the wall, compressing the spring, until the mass isin position .When you release the mass, the spring will exert a force, pushing the mass back until itreaches position , w.By that point, the spring will be stretched out, and will be exerting a force to pull the mass back in toward the wall. Because we are dealing with an idealized frictionless surface, the mass will not be slowed by the force of friction, and will oscillate back andforth repeatedly between an.This is all well and good, but we can’t get very far in sorting out the amplitude, the velocity, the energy, or anything else about the mass’s motion if we don’t understand the manner in which the spring exerts a force on the mass attached to it. The force, F, that the spring exerts on the mass is defined by Hooke’s Law:Simple Harmonic OscillationA mass oscillating on a spring is one example of a simple harmonic oscillator. Specifically, a simple harmonic oscillator is any object that moves about a stable equilibrium point and experiences a restoring force proportional to the oscillator’s displacement.For an oscillating spring, the restoring force, and consequently the acceleration, are greatest and positive at . Tan.There are a number of important properties related to the motion of a mass on a spring, all of which are fair game for SAT II Physics. Remember, though: the test makers have no interest in testing your ability to recall complex formulas and perform difficult mathematical operations. You may be called upon to know the simpler of these formulas, but not the complex ones. As we mentioned at the end of the section on pulleys, it’s less important that you memorize the formulas and more important that you understand what they mean. If you understand the principle, there probably won’t be any questions that will stump you.Period of OscillationThe period of oscillation, T, of a spring is the amount of time it takes for a spring to complete a round-trip or cycle. Mathematically, the period of oscillation of a simple harmonic oscillator described by Hooke’s Law is:) isis defined by:The potential energy of a spring is greatest when the coil is maximally compressed or stretched, and is zero at the equilibrium position.Kinetic EnergySAT II Physics will not test you on the motion of springs involving friction, so for the purposes of the test, the mechanical energy of a spring is a conserved quantity. As we recall, mechanical energy is the sum of the kinetic energy and potential energy.At the points of maximum compression and extension, the velocity, and hence the kinetic energy, is zero and the mechanical energy is equal to the potential energy, U s=1/.2You won’t need to know this equation, but it might be valuable to note that the velocity increases with a large displacement, a resistant spring, and a small mass.SummaryIt is highly unlikely that the formulas discussed above will appear on SAT II Physics. More likely, you will be asked conceptual questions such as: at what point in a spring’s oscillation is the kinetic or potential energy maximized or minimized, for instance. The figure below summarizes and clarifies some qualitative aspects of simple harmonic oscillation. Your qualitative understanding of the relationship between force, velocity, and kinetic and potential energy in a spring system is far more likely to be tested than your knowledge of the formulas discussed above.In this figure, v represents velocity, F represents force, KE represents kinetic energy, and represents potential energy.Vertical Oscillation of SpringsNow let’s consider a mass attached to a spring that is suspended from the ceiling. Questions of this sort have a nasty habit of coming up on SAT II Physics. The oscillation ofthe spring when compressed or extended won’t be any different, but we now have to take gravity into account.Equilibrium PositionBecause the mass will exert a gravitational force to stretch the spring downward a bit, the equilibrium position will no longer be at x = 0, but at x = –h, where h is the vertical displacement of the spring due to the gravitational pull exerted on the mass. The equilibrium position is the point where the net force acting on the mass is zero; in other words, the point where the upward restoring force of the spring is equal to the downward gravitational force of the mass.Combining the restoring force, F = –kh, and the gravitational force, F = mg, we can solve for h:an.Throughout the motion of the mass, the force of gravity is constant and downward. The restoring force of the spring is always upward, because even atthewoan.where is g is the spring’s (elastic) potential energy.Note that the velocity of the block is zero at an, aan angreatest when the spring is maximally extended at anexceptions. For instance, a plucked guitar string releases sound energy: the music we hear is the result of the string returning to its equilibrium position.The movement of an oscillating body is called harmonic motion. If you were to graph the position, velocity, or acceleration of an oscillating body against time, the result would be a sinusoidal wave; that is, some variation of a y = a sin bx or a y = a cos bx graph. This generalized form of harmonic motion applies not only to springs and guitar strings, but to anything that moves in a cycle. Imagine placing a pebble on the edge of a turntable, and watching the turntable rotate while looking at it from the side. You will see the pebble moving back and forth in one dimension. The pebble will appear to oscillate just like a spring: it will appear to move fastest at the middle of its trajectory and slow to a halt and reverse direction as it reaches the edge of its trajectory.This example serves two purposes. First, it shows you that the oscillation of springs is just one of a wide range of phenomena exhibiting harmonic motion. Anything that moves in a cyclic pattern exhibits harmonic motion. This includes the light and sound waves without which we would have a lot of trouble moving about in the world. Second, we bring it up because SAT II Physics has been known to test students on the nature of the horizontal or vertical component of the motion of an object in circular motion. As you can see, circular motion viewed in one dimension is harmonic motion.Though harmonic motion is one of the most widespread and important of physical phenomena, your understanding of it will not be taxed to any great extent on SAT II Physics. In fact, beyond the motion of springs and pendulums, everything you will need to know will be covered in this book in the chapter on Waves. The above discussion is mostly meant to fit your understanding of the oscillation of springs into a wider context.。
SAT2 物理真题 7套
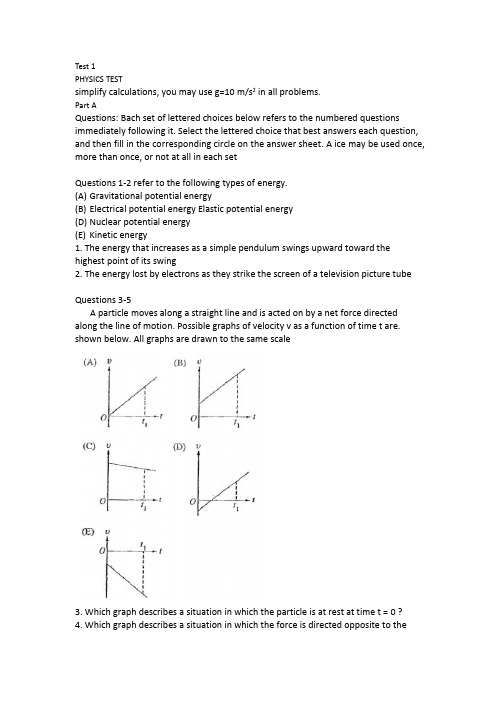
Test1PHYSICS TESTsimplify calculations,you may use g=10m/s2in all problems.Part AQuestions:Bach set of lettered choices below refers to the numbered questions immediately following it.Select the lettered choice that best answers each question, and then fill in the corresponding circle on the answer sheet.A ice may be used once, more than once,or not at all in each setQuestions1-2refer to the following types of energy.(A)Gravitational potential energy(B)Electrical potential energy Elastic potential energy(D)Nuclear potential energy(E)Kinetic energy1.The energy that increases as a simple pendulum swings upward toward the highest point of its swing2.The energy lost by electrons as they strike the screen of a television picture tubeQuestions3-5A particle moves along a straight line and is acted on by a net force directed along the line of motion.Possible graphs of velocity v as a function of time t are. shown below.All graphs are drawn to the same scale3.Which graph describes a situation in which the particle is at rest at time t=0?4.Which graph describes a situation in which the force is directed opposite to theparticle's velocity for the entire time between t=0and t=t1?5.Which graph indicates the least magnitude of acceleration for the particle?Questions6-8Diagram below shows pan of a transverse wave on a siring at one instant of time. The period of the wave and the wavelength isλ.The wave is traveling to the right.A.Point AB.Point BC.Point CD.Point DE.Point E1.Which point represents a distance of one wavelength from the origin?2.Which point is al a distance from the origin equal to the distance traveled by thewave in line2T(two periods)?3.Which point is山e siring moving upward at the instant shown?Questions9-11The following diagrams each show an object of mass m moving with instantaneous velocity v.In each case,F is the applied force,and f is the frictional force exerted on the object.In case I,the object moves in a circle which constant speed.(A)None(B)I only(C)II only(D)III only(E)I,II,and III9.In which of these cases are the velocity and the acceleration in the same direction at the instant shown?10.In which of these casts are the velocity and the acceleration in oppositedirections at the instant shown?11.In which of these cases are the velocity and the net force perpendicular to each other at the instant shown?Questions12-13Each of the five diagrams below represents an electron e that moves in the plane of the page into a uniform magnetic field as shown.The symbol represents a magnetic field directed into the plane of the page and the symbol O represents a magnetic field directed out of the plane of the page,the parallel lines represent magnetic fields in the directions indicated by the arrows.12.In Which diagram will the magnetic force on the electron be zero?13.In which diagram will the magnetic force on the electron be in the plane of thepage and directed initially toward the lop of the page?Part B14.A block of mass M is moving to die right,pilled across a floor by a horizontal force P.The force due to friction is f,the normal force is N,and the acceleration due to gravity is g.Which of the fallowing diagrams best shows the forces acting on block?15.A2,000-kilogram dump truck is initially e on a level surface at a constant speedof20per second.As the truck coasts under a lot ramp,a500-Jdlograni load is dropped only the speed of the truck and load immediate afterward is(A)5m/s(B)16m/s(C)20m/s(D)26m/s(E)50m/s16.Two airplanes are flying at the same height and with the same speed v.With respect ground,plane X is flying due east and plan flying due north.The relative velocity of plan as observed from plane X is in what direct(A)Northwest(B)Northeast(C)Southeast(D)North(E)East17.A20kilogram mass is accelerated on a hone surface at the rate of2meters persecond in the direction of motion by application of50newton force.What is the frictional force on the mass?(A)2N(B)10N(C)20N(D)30N(E)40N18.Which of the following devices,when used separately,can spread out white lightinto its component wavelengths?I.A diffraction gratingII.A prismIII.A front-surface mirror(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III19.A tree of height25meters is photographed by a person standing100metersfrom the tree.The film on which the image is formed is0.10meter from the lens.Which of the following is most nearly the height of the image of the tree on the film?(A)0.001m(B)0.025m(C)0.10m(D)0.25m(E)0.40m20.Unpolarized light is passed through two ideal polarizers,as shown above.Thelight passes first through the polarizer whose axis of polarization is vertical;next it passes through the polarizer whose axis of polarization is horizontal.Which of the following correctly describes bow light would emerge from the secondpolarizer?(A)It would be unpolarized.(B)It would be polarized vertically.(C)It would be polarized horizontally.(D)It would be polarized at45°to the vertical.(E)No light would emerge from the second21.Power plants give off waste heat to the environment.Which of the following combinations of power plant input and efficiency would result in the most waste heal?Input Efficiency(A)1,000MW30%(B)1,000MW40%(C)900MW30%(D)900MW40%(E)800MW35%22.A sample of a particular substance has a mass of0.500kilogram.If1,000joules of heat energy is required to increase the temperature of the sample by1.00°C,what is the specific heat of the substance?(A)2,000J/kg·C°(B)1,000J/kg·C°(C)500J/kg·C°(D)250J/kg·C°(E)Zero23.A snail moves along a straight,level sidewalk.The first0.5hour it travels2.0meters,and the next2.5hours it travels an additional4.0meters.What is the average speed of the snail over this3-hour period?(A)2.0m/hr(B)2.8m/hr(C)3.0m/hr(D)4.5m/hr(E)5.6m/hr24.A block of mass m is pulled across a horizontal floor by the force F,as shown above.The coefficient of friction between the block and the floor is.Which of the following is an expression for the frictional force excited on the block?(A)μmg(B)μF(C)μmg-F(D)μmg+F(E)μ(mg-F)25.An object of mass2kilograms falls from a tabletop1meter above the floor Air resistance is negligible.Which of the following quantities decreases during the object's fall?(A)Magnitude of acceleration(B)Magnitude of momentum(C)Speed(D)Kinetic energy(E)Potential energy26.Electromagnetic waves and sound waves have all of the following characteristics in common EXCEPT:(A)They can be absorbed.(B)They can be reflected.(C)They can exhibit interference.(D)They require air or another material medium in which to propagate.(E)Their velocities of propagation depend on the medium through which they arepropagating.27.The diagram above shows lines of electric field in the vicinity of two objectshaving charges Q1and Q2.From the diagram one cap infer that(A)Q1and Q2are of approximately equal magnitude and of the same sign(B)Q1and Q2are of approximately equal magnitude and of opposite sign(C)Q1and Q2are of very unequal magnitudeand of the same sign(D)Q1and Q2are of very unequal magnitudeand of opposite sign(E)another charged object must be nearby29.The half-life of radioactive polonium218is-about3minutes.A1-gram sample of this isotope h prepared and its activity determined at3-minute intervals.The amount of polonium218expected to remain12minutes after preparation is(A)1/2gram(B)1/4gram(C)1/8gram(D)1/12gram(E)1/16gram30.Four spaceships pass an asteroid at the relative velocities shown in the figure above.The ships arc all moving parallel to the length of the asteroid.A person on each of the ships and one on the asteroid measure the length L of the asteroid.The longest measurement is obtained by the person at which location?(A)The asteroid(B)Ship B(C)Ship C(D)Ship D(E)Ship E31.All of the following are examples of fractal patterns EXCEPT(A)branching sections of broccoli(B)cracks in dried mud(C)the structure of air passages in the lungs(D)frost patterns on a window(E)the shape of an insect32.Electric charge can be stored in a(A)capacitor(B)generator(C)motor(D)resistor(E)transformer33.A positively changed particle enters a region of uniform electric field betweentwo plates charged as shown above.If the particle is originally aimed at midpoint between the plates,it will be(A)undeflected(B)deflected toward plate I along a parabolic path(C)deflected toward plate I along a circular path(D)deflected toward plate II along a parabolic path(E)deflected toward plate II along a circular path34.A gas is confined in a cylinder with a piston that may be pushed in or pulled out.It is also possible to add heat to or remove heat from the gas independently of the piston motion.Which of the following combinations of piston motion and independent heat transfer is certain to increase the temperature of the gas?Piston Motion Heat(A)No piston motion Removed from the gas(B)Pulled oat None transferred(C)Pulled out Added to the gas(D)Pushed in Removed from the gas(E)Pushed in Added to the gas35.A lightbulb on a thermally insulated stand is place as shown above in a closed container from which ills air is then completely removed.When the but is lit,heat can be transferred through the space between the bulb and the walls of the container by which of the following?I.ConductionII.ConvectionIII.Radiation(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III36.A large square sheet of metal has a square hole cut in it.When the temperatureof the sheet is decreased,the area of the sheet will decrease.What will happen to the area of the bole?(A)It will not change.(B)It will always increase.(C)It will always decrease.(D)It will decrease only if the hole is not in the exact center of the sheet.(E)It will increase only if the hole is in the exact center of the sheet.37.If the density of See is0.90gram par cubic cent meter,then the volume of ice that will result from the freezing of1.0gram of water is most nearly(A)0.1cm3(B)0.9cm3(C)1.0cm3(D)1.1cm3(E)1.9cm3Questions38-40A car moves along a straight,level road with a constant velocity of20m/s for1 minute,then slows down uniformly and conies to rest over the course of another1 minute.Assume that the direction of motion is the positive direction.38.Which of the following graphs best represents the velocity v of the car versustime t for the2minutes that the car was in motion?39.Which of the following graphs best represents the acceleration a of the carversus time t for the2minutes that the car was in motion?40.The magnitude of the acceleration of the car during the second minute is most nearly(A)0.017m/s2(B)0.033m/s2(C)0.33m/s2(D)10m/s2(E)20m/s241.An object with mass2kilograms is dropped from n height of6meters.Assumingthat air resistance is negligible,the kinetic energy of the object at the instant before it hits the ground is most nearly(A)0(B)12J(C)30J(D)120J(E)300J42.Two blocks,of mass m1and m2,receptivity,are released simultaneously from the same height,fall freely with negligible air resistance.While the blocks fail,what is the ratio(A)1(B)m1(C)m(D)(m1m2)2(E)m2m143.Which of the following factors will most likely have the LEAST effect on thehorizontal range of a stone that is thrown near the surface of Earth?(A)The direction of the wind(B)The angle above the horizontal al which the stone is thrown(C)The initial vertical height of the stone above its landing point(D)The variation in the gravitational field over the path of the stone(E)The shape of the stone44.A satellite of mass m orbits the Earth of mass.If G is the constant in Newton’sJaw of universe gravitation,what is the resultant force on the satellite thereference frame in which the satellite moves in a circular orbit of radius r?(A)It has magnitude GmM r2and is directed inward,toward the center of the orbit.(B)It has magnitude GmM r2and is directed outward,away from the center of the or(C)It has magnitude mG and is directed inward toward the center of the orbit.(D)It has magnitude mG and is directed outward,away from the center of the orbit.(E)It is zero.45.A constant net force of magnitude F accelerate a block of mass m from rest atposition l to2speed v at position2,which is a distance from position1,as shown above.Expressions are equal to the work done by F in moving block fromposition1to position2include w of the following?I.12mv2II.MgdIII.Fd(A)I only(B)II only(C)I and III only(D)II and III only(E)I,II,and III46.The figures below show wires of various shapes whose ends arc connected to thepositive(+)and negative(-)terminals of a battery and a plane that isperpendicular to the vertical wires.In which figure is the magnetic field in the given plane correctly shown by the arrows?47.In the circuit shown above,the potential difference across either of the2-ohmresistors is(A)0(B)13V(C)3V(D)4V(E)5V48.Particles I,II,and III have electric charges of equal magnitude and are evenlyspaced along a line.Their signs are as shown above.The particles arc subject only to the forces exert on each other due to their electric charges.A net force10the 3eft acts on which of the particles?(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III49.The resistance of a copper wire depends on which of the following?I.The length of the wireII.The cross-sectional area of the wireIII.The temperature of the wire(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III50.A permanent magnet is in the vicinity of a coil of conducting wire.Which of the following is required to produce an induce an current in the coil?(A)The magnet and the coil must be touching each other.(B)The magnet must be inside the coil.(C)The magnet and coil must both be at rest.(D)The magnet and coil must be moving with the same velocity.(B)The magnet and the coil must be moving relative to each other.51.Which of the following always occurs when an atom emits electromagneticradiation?(A)The atomic number of the atom increases.(B)The atomic number of the atom decreases.(C)A new isotope is formed.(D)An electron jumps to a higher atomic energy level.(E)The total energy of the atom is reduced.52.An atom of one isotope of oxygen contains8protons and8neutrons.Which ofthe following could be the number of protons and neutrons in an atom ofanother isotope of oxygen?(A)6protons and10neutrons(B)7protons and10neutrons(C)8protons and9neutrons(D)9protons and7neutrons(E)9protons and8neutrons53.The equation x=x0+v0t+12at2,where v0and a are nonzero constants,is valid for giving the x-coordinate of an object as a function of time t in which of the following cases?(A)The object is sliding down a frictionless inclined plane.(B)The object is pulled by a constant force on a horizontal surface whose friction increases(C)The is oscillating on die end of a spring oriented in the x direction.(D)The object is moving at constant speed in a horizontal circle.(E)The object is accelerating down a circular54.A spaceship of mass m is traveling around a planet in a circular orbit that has a radius r.The gravitational force F on the spaceship is directed toward the planet.In this circular orbit,the speed of the spaceship is(A)mrF(B)rF/m(C)r2F/m(D)rF/m(E)r2F/m55.A large and a small ball,made of the same uniform density foam,are released atthe same time from point100meters above Earth.After1second the large ball is observed to have fallen farther than the small ball.Which of the followingexplains this observation?(A)The large hall weighs more than the small ball.(B)The gravitational force on the large ban is greater.(C)The buoyant force of the air on the large ball is greater.(D)The large ball has a greater ratio of mass to surface area.(E)Water vapor in the air adheres to the small ball more readily and thus increases its in?56.A child stands3.5meters in front of a plane mirror.An adult stands directlybehind the child,7meters in front of the mirror.How far is the adult from the image of the child?(A)2m(B)3.5m(C)4.5m(D)7m(E)10.5m57.Light waves in air are incident on a screen with H small hole in it and form adiffraction pattern on die other side.The diffraction pattern will spread over a larger area under which of the following circumstances?I.The wavelength of the waves is increased.II.The frequency of the waves is increased.III.The hole is made smaller.(A)I only(B)II only(C)I and II only(D)I and III only(E)I,II,and III58.All of the following are examples of areas in which Galileo made scientificcontributions EXCEPT the(A)development of the concept of inertia(B)determination that all objects fall with the same acceleration due to gravity(C)discovery of the moons of Jupiter(D)discovery that the universe is expanding(E)discovery of the craters6i the Moon59.Correct statements about Bohr's planetary model of the hydrogen atom includewhich of the following?I.No radiation is emitted while the electron remains in the same energy level. II.Emission or absorption of radiation accompanies die transition of the electron from one energy level to another.III.Visible light is emitted when the election is raised from a lower energy level to a higher energy level.(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III60.An experiment on the photoelectric effect is conducted with a sheet of zinc onwhich ultraviolet light is shone.The longest wavelength of the light that will cause photoelectrons to be emitted from the zinc surface is3.5x10-7meter.Which of the following is the best estimate of the minimum energy required to eject photoelectrons from zinc?(c=3x108m/s and h=4.14x10-15eV·s)(A)5x10-30eV(B)2x10-21eV(C)4eV(D)1x102eV(E)9x107eV61.In order to observe the Doppler effect,which of the following is necessary?(A)Longitudinal waves(B)Transverse waves(C)A medium moving with constant velocity(D)An observer and a source of waves stationary with respect to each other(E)An observer and a source of waves moving with respect to each other62.Light is incident on a flat piece of glass that is coated with a thin film as shownabove,and a person at point X observes both bright and dark bands in the film.Which of the following phenomena does NOT occur in the formation of these bands?(A)Reflection(B)Interference(C)Refraction(D)Diffraction(E)Phase change63.A scuba diver several feet underwater sees a bird perched on a raised cliff by theedge of the water.To the diver the bird appears to be(A)beneath the surface of the water(B)at the surface of the water(C)above the surface but below the actual position of the bird(D)at the actual position of the bird(E)above the actual position of the bird64.The system shown above consists of two positively charged particles,X and Y,adistance d apart.Particle X is fixed and particle Y is movable.The electricpotential energy of the system could be increased by which the following?I.Decreasing the charge on YII.Moving y to the rightIII.Moving Y to the left(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III65.The repulsive force acting on each of two small chained balls is12newtons whentheir centers arc separated by4centimeters,a distance much greater than the radii of the balls.If the balls are moved to a separation of8centimeters,therepulsive force is(A)3N(B)6N(C)12N(D)24N(E)48N66.A parallel-plate capacitor with capacitance C at plate separation d has a potentialdifference of across it.The magnitude of the electric field between its plates is(A)V d(B)CV d(C)Vd C(D)CV(E)Cd67.A100-grain sphere of iron at100℃is dropped into100grams of water at10℃.The water and iron quickly reach an equilibrium temperature much closer to 10℃than to100℃Which of the following correctly explains this phenomenon?(A)Iron is much denser than water,therefore the volumes of water in thisexperiment wax much greater than the volume of iron.(B)The specific heat of water is much greater than the specific heat of iron.(C)Water al10℃is ice,and all the ice had to the melted before(he temperatureof the wale could increase.(D)Because iron is solid at100℃,heat near the center of the sphere could not get into the water.(E)A large amount of heat was lost to the surroundings before the water and iron reached equilibrium.68.If an object moves in a circular path with a speed that increases at a constantrate,which of the following can be concluded?(A)The radial component of the force acting of the object is zero.(B)The net force acting on the object is zero.(C)The net force acting on the object is directed toward the center of the circle.(D)The net force acting on the object has a race component and a tangential component the are equal at all times.(E)The tangential component of the force acting on the object is constant.70.True statements about Jupiter as it moves in its elliptical orbit around the Sun include which of the following?I.It has its greatest speed when closest to the Sun.II.It bas its greatest potential energy when farthest from the Sun.III.The magnitude of its acceleration is constant.(A)I only(B)II only(C)I and II only(D)II and III only(E)I,II,and III71.A mass on an ideal spring oscillates with a period T,and its amplitude ofoscillation is A.If the amplitude is increased to4A,the period will be(A)T16(B)T2(C)T(D)2T(E)16TQuestions72-73An electric lightbulb marked“60W,120V”is connected to a l20-volt power line. The bulb stays lit for100seconds.72.How much electrical energy is converted into heat and light energy during thetime the bulb is lit?(A)60J(B)3,600J(C)6,000J(D)7,200J(E)12,000J73.What is the resistance of the wire in the lightbulb?(A)30ohms(B)60ohms(C)90ohms(D)240ohms(E)2,400ohms74.A5-kilogram block is initially at rest on a Action-less table.The graph aboveshows a constant force of200newtons that is applied for a short pencil of time on the block.What is the magnitude of the change in momentum of the block during the time that the force acts on it?(A)4kg·m/s(B)20kg·m/s(C)200kg·m/s(D)1,000kg·m/s(E)10,000kg·m/s75.The ratio of the Barth's radius to the Moon's distance from the Earth,R E/R M is1/60.If g is the acceleration due to gravity at the Earth’s surface and a is theacceleration at the distance R M due to the Barth's gravitational field,what is the ratio g/a?(A)6/1(B)10/1(C)60/1(D)120/1(E)3,600/1TEST2PART ADirections:Each set of lettered choices below refers to the numbered questions immediately following it.Select the one lettered choice that best answers each question,and then fill in the corresponding circle on the answer sheet.A choice may be used once,more than once,or not at all in each set.Questions1-2The figure above represents a track on which an object slides without friction. The object is released from the top of the track in the position shown.(A)Point A(B)Point B(C)Point C(D)Point D(E)Point£1.At which of the labeled points is the object's potential energy greatest?2.At which of the labeled points is the object's kinetic energy greatest? Questions3-6A person throws a ball with an initial speed v0from the deck of a house,in any one of the four initial directions shown in the diagrams above.In each case the ball is released at a height H above the ground.Air resistance is negligible.(A)A only(B)B only(C)C only(D)D only(E)It is the same for all four cases.3.In which case does the ball travel the greatest horizontal distance before hitting the ground?4.In which case does the ball have the greatest speed when it hits the ground?5.In which case does the ball have the greatest acceleration just before it hits the ground?6.In which case is the ball in the air for the longest time?Questions7-8refer to the following properties of light waves.I.FrequencyII.WavelengthIII.Speed(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III7.in a vacuum,all colors of light have the same value for which of the properties?8.When light passes from air into glass,which of the properties changes? Questions9-10A block on a friction less tabletop is attached to a horizontal spring that is fixed to a wall,as shown above.The block is pulled to the right.Choose answers to the following questions from the set of graphs below.9.Which graph represents the magnitude of the linear restoring force as a functionof the block's displacement from its equilibrium position?10.Which graph represents the elastic potential energy stored in the spring as afunction of the blocks displacement from equilibrium?Questions11-12A particle moves along the x axis and is acted on by a net force directed along the line of motion.Possible graphs of velocity v x as a function of time t are shown below.All graphs are drawn to the same scale.11.Which graph is NOT possible for a force that is constant in magnitude anddirection?12.Which graph describes a situation in which the velocity of the particle changesdirection between t=0and t=t1?Questions13-15The following diagrams each represent an isolated charge+Q at center of the circle shown.In each case,a test charge+q is moved from rest at X to rest at Y by an external force along the path shown.(A)I only(B)III only(C)I and II only(D)II and III only(E)I,II,and III13.The net work done by the external force is maximum in which of these cases?14.No net work is done by the external force in which of these cases?15.The electric potential at Y is higher than at X in which of these cases?Questions16-17The statements below describe experimental j observations of processes involving electrons.I.An electric current passes through a gas,and a characteristic spectrum isobserved.II.Electrons are scattered from the surface of a metal,and a diffraction pattern is observed.III.Electrons are dislodged from a metal surface that has been struck by photons, and the energy of the electrons is measured.(A)II only(B)III only(C)I and II only(D)I and III only(E)I,II,and III16.Evidence for the particle nature of light is provided by which of the observations?17.Evidence that an electron needs a minimum energy to leave a surface is provided by which of the observations?Questions18-19refer to the following types of images that may be formed w hen an object is placed in front of a lens or mirror.I.Real image,larger than the objectII.Real image,smaller than the objectIII.Virtual image(A)1only(B)III only(C)I and II only(D)II and III only(E)I,II,and III18.Which of these types of images can be formed by the convex mirror shownabove?。
SATOG全部十套题语法部分分析

C项错误,and连接的两个部分时态不一致。
D项错误,谓语动词改为现在分词形式主句就没有谓语了。
E项错误,语态有问题,不宜用被动。
翻译
W.S的三卷自传以他在尼日利亚小山村的童年开始,并随着他在非洲西部最好的大学——Ibadan的岁月达到高潮。
备注
题干
7.Dressed in a crisp. clean uniform. it reflected the efficient manner of the tour guideas she distributed maps for a walking tour of central Canberra.
解析
A项错误,that引导定语从句,which也引导定语从句,所以本句就没有谓语了。
B项正确,were caused by标准的被动形式做谓语。
C项错误,because引导原因状语从句,还是没有谓语,要把原句的that去掉,用occurred做主句的谓语。
D项错误,改变时态。
E项错误,occur和happen赘余。
E项错误,原因同C项,主句没有谓语了。
翻译
当她分发到堪培拉中心的徒步旅行的地图时,她清新,整洁的套装表现了导游的得体礼仪。
备注
题干
8. A cure for some kinds of cancer,scientists believe, may befound within the next decade.
(E)The crisp, clean uniform of the tour guide, a reflection of her efficient manner
2024届高考强基3套卷全国卷(二)物理高频考点试题

2024届高考强基3套卷全国卷(二)物理高频考点试题一、单项选择题(本题包含8小题,每小题4分,共32分。
在每小题给出的四个选项中,只有一项是符合题目要求的)(共8题)第(1)题升流器是电力部门和工矿企业的电器设备做电流负载试验及温升试验的专用设备,其工作原理简化如图所示。
某次外接调压器进行伏安特性试验,升流器第一级的自耦线圈滑头P位于线圈中点,为第二级升流器,均为理想变压器,不计线路电阻。
当采集负载R的电流值为输入端电流值的10倍时,则第二级升流器的匝数比为()A.1:5B.3:1C.4:1D.5:1第(2)题一根长度为L质量为m的粗细可忽略的导体棒A静止在一个足够长的光滑绝缘半圆柱体顶端,导体棒中通有垂直纸面向外大小为I的电流,其截面如图,现让导体棒由静止滑下,一段时间后从某一位置P离开半圆柱体。
若在圆心O处加一根垂直于截面通电情况与A相同的导体棒B(图中未画出),其它条件不变,则关于导体棒A离开半圆柱体的位置说法中正确的是( )A.可能在P的左上方B.可能仍在P处C.一定在P、Q之间某处D.可能在Q处第(3)题如图所示是大型游乐装置“大摆锤”的简图,摆锤a和配重锤b分别固定在摆臂两端,并可绕摆臂上的转轴O在纸面内转动。
若a、b到O的距离之比为2:1且不计一切阻力,则摆臂自由转动一周过程中( )A.a、b角速度之比为2:1B.摆臂对转轴的弹力保持不变C.摆臂对a和b作用力的功为0D.摆臂对a和b作用力的冲量为0第(4)题“拔火罐”是我国传统医学的一种治疗手段。
操作时,医生用点燃的酒精棉球加热一个小罐内的空气,随后迅速把小罐倒扣在需要治疗的部位,冷却后小罐便紧贴在皮肤上,如图所示。
小罐倒扣在身体上后,在罐中气体逐渐冷却的过程中,罐中气体质量和体积均可视为不变。
若罐中气体可视为理想气体,下列说法正确的是( )A.罐中气体的压强不变B.罐中气体的内能不变C.罐中气体分子的平均动能不变D.罐中单位体积内气体分子数不变第(5)题吊车是工程上常用的一种机械,如图所示为一吊车吊起一批木材,吊车大臂支架顶端固定一定滑轮,一根缆绳绕过定滑轮,一端固定于吊车车身的控制器上,另一端连接一吊钩,吊钩下挂着木材,保持缆绳的长度不变,控制吊车大臂支架绕固定轴转动使木材缓慢升高,在木材缓慢升高的过程中吊钩不会碰到定滑轮,木材也不会接触到大臂。
SAT2物理习题Waves

SAT2物理习题Waves以下是小编为大家整理的SAT2物理习题Waves,多做真题对sat物理成绩提高很有帮助,希望广大考生可以坚持每天做真题,赶快下载全篇吧。
1. What is the wavelength of a 5 Hz wave that travels with a speed of 10 m/s?(A) 0.25m(B) O.5m(C) 1m(D) 2m(E) 50m2. A rope of length 5 m is stretched to a tension of 80 N. If its mass is 1 kg, at what speed would a 10 Hz transverse wave travel down die string?(A) 2 m/s(B) 5 m/s(C) 20 m/s(D) 50 m/s(E) 200m/s3. A transverse wave on a long horizontal rope with a wavelength of 8 m travels at 2 m/s. At t = 0, a particular point on the rope has a vertical displacement of +A, where A is the amplitude of the wave. At what time will the vertical displacement of this same point on the rope be -A?(A) t = 1/8 s(B) t = 1/4 s(C) t = 1/2s(D) t = 2 s(E) t = 4s4. What is the wavelength of a wave with period 2s and speed 2 cm/s?(A) 0.25 cm(B) 0.5 cm(C) 1 cm(D) 2 cm(E) 4 cm5. A string, fixed at both ends, supports a standing wave with a total of 4 nodes. If the length of the string is 6 m, what is the wavelength of the wave?(A) 0.67 m(B) 1.2 m(C) 1.5 m(D) 3 m(E) 4 m6. A string, fixed at both ends, has a length of 6 m and supports a standing wave with a total of 4 nodes. If a transverse wave can travel at 40 m/s down the rope, what is the frequency of this standing wave?(A) 6.7 Hz(B) 10.0 Hz(C) 13.3 Hz(D) 20.0 Hz(E) 26.7 Hz7. A sound wave travels through a metal rod with wavelength λ and frequency ƒ. Which of the following best describes the wave when it passes into the surrounding air?Wavelength Freaueoey(A) Less than λ Equal to ƒ(B) Less than λ Less than ƒ(C) Greater than λ Equal to ƒ(D) Greater than λ Less than ƒ(E) Greater than λ Greater than ƒ8. In the figure below, two speakers, S1, and S2, emit sound waves of wavelength 2 m, in phase with each other.Let AP be the amplitude of the resulting wave at point P, and AQ the amplitude of the resultant wave at point Q. How does AP compare to AQ?(A) AP < AQ(B) AP = AQ(C) AP > AQ(D) AP < 0, AQ > 0(E) AP and AQ vary with time, so no comparison can be made.9. An observe mice of sound waves.By how much will the sound level decrease if the observer moves to a distance of 20 m ?(A) 1dB(B) 2dB(C) 10 dB(D) 18 dB(E) 20 dB10. An organ pipe that’s closed at one end has a length of 17 cm. If the speed of sound through the air insi de is 340 m/s, what is the pipe’s fundamental frequency?(A) 250 Hz(B) 500 Hz(C) 1,000 Hz(D) 1,500 Hz(E) 2,000 Hz11. A bat emits a 40 kHz “chirp” with a wavelength of 8.75 mm toward a tree and receives an echo 0.4 s later. How far is the bat from the tree?(A) 35 m(B) 70 m(Q 105 m(D) 140 m(E) 175 m12. A car is traveling at 20 m/s away from a stationary observer. If the car’s horn emits a frequency of 600 Hz, what frequency will the observer hear? (Use v = 340 m/s for the speed of sound.)(A) (34/36X600 Hz)(B) (34/32X600 Hz)(C) (36/34X600 Hz)(D) (32/34X600 Hz)(E) (32/36X600 Hz)。
2024届高考强基3套卷全国卷(二)物理试题

2024届高考强基3套卷全国卷(二)物理试题一、单选题 (共6题)第(1)题图甲是某人在湖边打水漂的图片,石块从水面弹起到触水算一个水漂,若石块每次从水面弹起时速度与水面的夹角均为,速率损失。
图乙是石块运动轨迹的示意图,测得石块打第一个水漂在空中的时间为,已知石块在同一竖直面内运动,当触水速度小于时石块就不再弹起。
不计空气阻力,重力加速度,石块在湖面上能漂起的次数为( )A.B.C.D.第(2)题老师做了一个物理小实验让学生观察:一轻质横杆两侧各固定一金属环,横杆克绕中心点自由转动,老师拿一条形磁铁插向其中一个小环,后又取出插向另一个小环,同学们看到的现象是()A.磁铁插向左环,横杆发生转动B.磁铁插向右环,横杆发生转动C.无论磁铁插向左环还是右环,横杆都不发生转动D.无论磁铁插向左环还是右环,横杆都发生转动第(3)题如图所示,质量为m的长木板放在水平地面上,站在木板上的人用斜向右下方的力F推箱子,三者都保持静止。
人和箱子的质量也均为m,重力加速度为g。
下列说法正确的是( )A.人对长木板的压力大小为mgB.长木板对地面的压力大于3mgC.箱子受到的摩擦力的方向水平向左D.地面对长木板的摩擦力的方向水平向左第(4)题一小球做平抛运动,关于小球的速度、动量、动能、重力势能的变化率随时间变化的图线,正确的是()A.B.C.D.第(5)题如图所示,木板B固定在弹簧上,木块A叠放在B上,A、B相对静止,待系统平衡后用竖直向上的变力F作用于A,使A、B一起缓慢上升,AB不分离,在A、B一起运动过程中,下面说法正确的是( )A.一起缓慢上升过程中A对B的摩擦力不变B.在某时刻撤去F,此后运动中A可能相对B滑动C.在某时刻撤去F,此后运动中AB的加速度可能大于gD.在某时刻撤去F,在A、B下降的过程中,B对A的作用力一直增大第(6)题2023年4月29日至5月3日,中国国际新能源、智能汽车博览会在石家庄举办,新能源是指刚开始开发利用或正在积极研究、有待推广的能源,如太阳能、风能和核聚变能等。
AP考试物理真题试卷

AP考试物理真题试卷一、选择题(共30分)1. 一个物体的质量为2千克,受到一个力F=10牛顿。
根据牛顿第二定律,这个物体的加速度是多少?A. 5 m/s²B. 10 m/s²C. 20 m/s²D. 50 m/s²2. 一个在水平面上的物体,受到一个水平向右的力F=20牛顿,同时受到一个摩擦力f=10牛顿。
如果物体的质量为5千克,求物体的加速度。
A. 2 m/s²B. 4 m/s²C. 6 m/s²D. 8 m/s²3. 一个物体从静止开始,以恒定加速度a=3 m/s²加速运动,经过5秒后的速度是多少?A. 7.5 m/sB. 10 m/sC. 15 m/sD. 20 m/s4. 一个物体以初速度v₀=10 m/s在水平面上运动,受到一个与运动方向相反的摩擦力f=5牛顿。
如果物体的质量为3千克,求物体在5秒内的位移。
A. 25 mB. 37.5 mC. 50 mD. 75 m5. 一个质量为m的物体在竖直方向上受到重力作用,同时受到一个向上的拉力T。
如果物体保持静止,那么拉力T与重力mg的关系是什么?A. T = mgB. T = 0C. T < mgD. T > mg二、计算题(共40分)6. 一个质量为4千克的物体在光滑水平面上受到一个水平力F=30牛顿的作用。
求物体在3秒内的位移和最终速度。
7. 一个质量为6千克的物体从高度h=10米处自由落体,忽略空气阻力。
求物体落地时的速度和落地前2秒内的位移。
8. 一个物体在水平面上以初速度v₀=15 m/s开始运动,受到一个与运动方向相反的摩擦力f=20牛顿。
求物体在5秒内的平均速度和位移。
9. 一个物体在竖直平面内做抛物线运动,初速度v₀=20 m/s,抛射角度为45度。
求物体在水平方向和竖直方向上的位移,以及运动时间。
10. 一个质量为8千克的物体在竖直方向上受到一个向上的拉力T=50牛顿,同时受到重力作用。
act初高中物理试题及答案

act初高中物理试题及答案一、选择题(每题3分,共30分)1. 光在真空中的传播速度是()。
A. 300,000 km/sB. 299,792,458 m/sC. 3.0 x 10^8 m/sD. 3 x 10^5 km/s2. 牛顿第一定律描述的是()。
A. 物体在没有外力作用下的运动状态B. 物体在受到外力作用下的运动状态C. 物体在受到摩擦力作用下的运动状态D. 物体在受到重力作用下的运动状态3. 以下哪个选项是正确的能量转换形式?()A. 电能转化为化学能B. 化学能转化为电能C. 电能转化为热能D. 所有选项都是正确的4. 一个物体的质量是2千克,当它受到10牛顿的力时,它的加速度是多少?()A. 5 m/s²B. 10 m/s²C. 20 m/s²D. 无法确定,因为缺少距离信息5. 以下哪个选项是电磁波谱中的一部分?()A. 无线电波B. 可见光C. X射线D. 所有选项都是正确的6. 以下哪个选项不是热力学第一定律的组成部分?()A. 能量守恒B. 能量转换C. 能量的产生D. 能量的消耗7. 一个物体在水平面上以恒定速度移动,以下哪个力是不需要考虑的?()A. 重力B. 摩擦力C. 推力D. 空气阻力8. 以下哪个选项是正确的波长和频率的关系?()A. 波长和频率成正比B. 波长和频率成反比C. 波长和频率无关D. 波长和频率相等9. 一个完全弹性碰撞中,以下哪个物理量是守恒的?()A. 动能B. 动量C. 总能量D. 机械能10. 以下哪个选项是正确的功率定义?()A. 功率是单位时间内所做的功B. 功率是单位时间内消耗的能量C. 功率是单位时间内产生的热能D. 功率是单位时间内转移的电荷二、填空题(每题2分,共20分)1. 根据欧姆定律,电流I与电压V和电阻R之间的关系是:I =________。
2. 牛顿第二定律的公式是:F = ________。
SAT物理模拟试题

SAT模拟试题(1)1.An object that's moving with constant speed travels once around a circular path。
True statements about this motion include which of the following?A.The displacement is zeroB. The average speed is zeroC. The acceleration is zero D。
None of the aboveA2.Which of the following must always be true?1.If an object’s acceleration is constant,then it must move in a straight line2。
If an object’s acceleration is zero,then its speed must remain constant3.If an object's speed remains constant,then its acceleration must be zero。
A.1 and 2 only B。
1 and 3 only C.2 only D。
3 only E.2 and 3 onlyC4.A frictionless inclined plane of length 20m has a maximum vertical height of 5m。
If an object of mass 2 kg is planced on the plame . Which of the following best approximates the net force it feels?A.5N B。
10N C。
SAT2物理习题Newton’sLaws

SAT2物理习题Newton’sLawsSAT2物理习题Newton’s Laws以下是⼩编为⼤家整理的SAT2物理习题Newton’s Laws,希望对⼤家有帮助,跟随SAT资料下载的⼩编⼀起来练习练习吧! SAT2物理习题Newton’s Laws1. A person standing on a horizontal floor feels two forces: the downward pull of gravity and the upward supporting force from the floor. These two forces(A) have equal magnitudes and form an action/reaction pair(B) have equal magnitudes but do not form an action/reaction pair(C) have unequal magnitudes and form an action/reaction pair(D) have unequal magnitudes and do not form an action/reaction pair(E) None of the above2. A person who weighs 800 N steps onto a scale that is on the floor of an elevator car. If the elevator accelerates upward at a rate of 5 m/s2, what will the scale read?(A) 400N(B) 800N(C) 1000N(D) 1200N(E) 1600N3. A firicdonless inclined plane of length 20 m has a maximum vertical height of 5 m. If an object of mass 2 kg is placed on the plane, which of the following best approximates the net force it feels?(A) 5 N(B) 10 N(C) 15 N(D) 20 N(E) 30 N4. A 20 N block is being pushed across a horizontal table by an 18 N force. If the coefficient of kinetic friction between the block and the table is 0.4, find the acceleration of the block.(A) 0.5 m/s2(B) 1 m/s2(C) 5 m/s2(D) 7.5 m/s2(E) 9 m/s25. The coefficient of static friction between a box and a ramp is 0.5. The ramp’s incline angle is 30°. If the box is placed at rest on the ramp, the box will(A) accelerate down the ramp(B) accelerate briefly down the ramp but then slow down and stop(C) move with constant velocity down the ramp(D) not move(E) Cannot be determined from the information given6.Assuming a Motionless, massless pulley, determine the acceleration of the blocks once they are released from rest7. If all of the forces acting on an object balance so that the net force is zero, then(A) the object must be at rest(B) the object’s speed will decrease(C) the object will follow a parabolic trajectory(D) the object’s direction of motion can change, but cannot its speed(E) None of the above8. A block of mass m is at rest on a frictionless, horizontal table placed in a laboratory on the surface of the earth. An identical block is at rest on a frictionless, horizontal table placed on the surfece of the moon. Let F be the net force necessary to give the earth-bound block an acceleration of a across the table. Given that gmoon is one sixth of gearth, the force necessary to give the moon-bound block the same acceleration a across the table is(A) F/12(B) F/6(C) F/3(D) F(E) 6F9. A crate of mass 100 kg is at rest on a horizontal floor. The coefficient of static friction between the crate and the floor is 0.4, and the coefficient of kinetic friction is 0.3. A force F of magnitude 344 N is then applied to the crate, parallel to the floor. Which of the following is true?(A) The crate will accelerate across the floor at 0.5 m/s2(B) The static friction force, which is the reaction force to F as guaranteed by Newton’s third law, will also have a magnitude of 344 N.(C) The crate will slide across the floor at a constant speed of 0.5 m/s(D) The crate will not move.(E) None of the above10. Two crates are stacked on top of each other on a horizontal floor; crate #1 is on the bottom, and crate #2 is on the top. Both crates have the same mass. Compared with the strength of the force F1 necessary to push only crate #1 at a constant speed across the floor, the strength of the force F2 necessary to push the stack at the same constant speed across the floor is greater than F1, because(A) the force of the floor on crate #1 is greater(B) the coefficient of kinetic friction between crate #1 and the floor is greater(C) the force of kinetic friction, but not the normal force, on crate #1 is greater(D) the coefficient of static friction between crate #1 and the floor is greater(E) the weight of crate #1 is greater11. An object moves at constant speed in a circular path. Which of the following statements is/are true?I. The velocity is constant.II. The acceleration is constant.III. The net force on the object is zero since its speed is constant(A) II only(B) I and III only(C) II and III only(D) I and II only。
2021CSHSAT基础知识调研答案物理1

2021CSHSAT基础知识调研性模拟测试(1)物理学科试题范围:新高考改革地区全部内容参考答案与试题解析一、选择题(共8小题,每小题6分,满分48分)1.(6分)2018年11月16日,第26届国际计量大会通过“修订国际单位制”决议,正式更新包括国际标准质量单位“千克”在内的4项基本单位定义,新国际单位体系将于2019年5月20日世界计量日起正式生效。
其中,千克将用普朗克常量(h)定义;安培将用电子电荷量(e)定义。
以基本物理常数定义计量单位,可大大提高稳定性和精确度。
关于普朗克常量和电子电荷量的单位,下列正确的是()A.普朗克常量的单位为kg•m3s﹣2B.普朗克常量的单位为kg﹣1•m2s﹣1C.电子电荷量的单位为A•sD.电子电荷量的单位为A•s﹣1【分析】根据公式h=Pλ,与q=It,结合动量P,波长λ,电流I,时间t的单位,从而即可求解。
【解答】解:根据公式h=Pλ,因动量P的单位为kg•m/s,波长λ的单位为m,那么普朗克常量h的单位为kg•m2/s再由q=It,结合电流I与时间t的单位,可知,电子电荷量q的单位为A•s,故ABD错误,C正确;故选:C。
【点评】本题考查磁场强度的单位,关键是结合具体公式进行分析,注意物理公式确定了各个物理量的单位关系,基础题目。
2.(6分)如图所示是氢原子的能级图,大量处于n=5激发态的氢原子向低能级跃迁时()A.一共能辐射6种频率的光子B.能辐射出3种能量大于10.2eV的光子C.能辐射出3种能量大于12.09eV的光子D.能辐射出能量小于0.31eV的光子【分析】依据数学组合公式,确定大量氢原子跃迁种类,再结合释放光子的能量即为两能级间的能级差,从而即可一一求解。
【解答】解:A、依据=10,可知,大量处于n=5激发态的氢原子向低能级跃迁时,共有10种不同频率的光子,故A错误;BCD、根据玻尔理论,从n=2能级跃迁到基态释放光子的能量:△E1=E2﹣E1=﹣3.4eV ﹣(﹣13.6eV)=10.2eV,从n=3能级跃迁到n=1能级释放光子的能量:△E2=E3﹣E1=﹣1.51eV﹣(﹣13.6eV)=12.09eV,从n=4能级跃迁到n=1能级释放光子的能量:△E3=E4﹣E1=﹣0.85eV﹣(﹣13.6eV)=12.75eV,从n=5能级跃迁到n=1能级释放光子的能量:△E4=E5﹣E1=﹣0.54eV﹣(﹣13.6eV)=13.06eV,从n=5能级跃迁到n=4能级释放光子的能量:△E5=E5﹣E4=﹣0.54eV﹣(﹣0.85eV)=0.31eV,由上分析,可知,辐射出的能量大于10.2eV的光子有3种,辐射出的能量大于12.09eV 的光子有2种,辐射出的光子能量至少等于0.31eV,故B正确,CD错误。
2024届高考强基3套卷全国卷(二)物理试题

2024届高考强基3套卷全国卷(二)物理试题一、单选题 (共6题)第(1)题如图是位于锦江乐园的摩天轮,高度为108 m,直径是98 m.一质量为50 kg的游客乘坐该摩天轮做匀速圆周运动旋转一圈需25 min.如果以地面为零势能面,则他到达最高处时的(取g=10 m/s2)( )A.重力势能为5.4×104J,角速度为0.2 rad/sB.重力势能为4.9×104J,角速度为0.2 rad/sC.重力势能为5.4×104J,角速度为4.2×10-3rad/sD.重力势能为4.9×104J,角速度为4.2×10-3rad/s第(2)题秦山核电基地共有9台核电机组,总装机容量是656.4万千瓦,年发电量约500亿千瓦时,其中包括中国自行设计、建造和运营管理的第一座30万千瓦压水堆机组。
设一吨标准煤可以发电3000度,每户人家平均每月用电200度。
根据以上信息,以下分析正确的是( )A.所用核燃料每年减轻的质量大约为B.秦山核电站每年发电量可以为户家庭供电一年C.秦山核电站利用核聚变产生的巨大核能D.秦山核电站的年发电量相当于少燃烧约吨标准煤第(3)题我国计划在2024年上半年发射“嫦娥六号”,实施月球背面采样。
若未来我国在月球发射一颗月球的同步卫星,已知月球的质量为,半径为,月球自转的角速度为,引力常量为,则月球同步卫星到月球表面的高度为( )A.B.C.D.第(4)题如图所示,甲、乙为长度、半径、材料均相同的质量分布均匀的圆柱体和半圆柱体,甲表面光滑,乙表面粗糙,半圆柱体乙的重力为G,两物体靠在一起放置在粗糙水平桌面上。
现过圆柱体甲的轴心施加一个始终与水平方向夹角斜向右上方的作用力F,将圆柱体甲缓慢地拉至圆柱体乙的顶端,乙始终处于静止状态。
在甲缓慢移动的过程中,下列判断正确的是( )A.两圆柱体间的压力由逐渐增大至B.拉力由逐渐减小至C.桌面对圆柱体乙的摩擦力逐渐减小D.桌面对圆柱体乙的支持力不变第(5)题如图甲所示,光滑水平地面上有A、B两物块,质量分别为2kg、6kg,B的左端拴接着一劲度系数为的水平轻质弹簧,它们的中心在同一水平线上。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
SAT物理考试-Inclined Planeswe know that when people are placed on slippery inclines, they slide down the slope. We also know that slides can sometimes be sticky, so that when you are at the top of the incline, you need to give yourself a push to overcome the force of static friction. As you descend a sticky slide, the force of kinetic friction opposes your motion. In this section, we will consider problems involving inclined planes both with and without friction. Since they’re simpler, we’ll begin with frictionless planes.Suppose you place a 10 kg box on a frictionless 30º inclined plane and release your hold, allowing the box to slide to the ground, a horizontal distance of d meters and avertical distance of h meters.Before we continue, let’s follow those three important preliminary steps for solving problems in mechanics:1. Ask yourself how the system will move: Because this is a frictionless plane,there is nothing to stop the box from sliding down to the bottom. Experience suggests that the steeper the incline, the faster an object will slide, so we can expect the acceleration and velocity of the box to be affected by the angle of the plane.2. Choose a coordinate system: Because we’re interested in how the box slidesalong the inclined plane, we would do better to orient our coordinate system to the slope of the plane. The x-axis runs parallel to the plane, where downhill is the positive xdirection, and the y-axis runs perpendicular to the plane, where up is the positive ydirection.3. Draw free-body diagrams: The two forces acting on the box are the force ofgravity, acting straight downward, and the normal force, acting perpendicular to theinclined plane, along the y-axis. Because we’ve oriented our coordinate system to the slope of the plane, we’ll have to resolve the vector for the gravitational force, mg, into its x- and y-components. If you recall what we learned about vector decomposition inChapter 1, you’ll know you can break mg down into a vector of magnitude cos 30º in the negative y direction and a vector of magnitude sin 30º in the positive x direction. The result is a free-body diagram that looks something like this:Decomposing the mg vector gives a total of three force vectors at work in thisdiagram: the y -component of the gravitational force and the normal force, which cancel out; and the x -component of the gravitational force, which pulls the box down the slope. Note that the steeper the slope, the greater the force pulling the box down the slope.Now let’s solve some problems. For the purposes of these problems, take theacceleration due to gravity to be g = 10 m/s 2. Like SAT II Physics, we will give you the values of the relevant trigonometric functions: cos 30 = sin 60 = 0.866, cos 60 = sin 30 = 0.500.1. What is the magnitude of the normal force?2. What is the acceleration of the box?3. What is the velocity of the box when it reaches the bottom of the slope?4.What is the work done on the box by the force of gravity in bringing it to the bottom of the plane?1. What is the magnitude of the normal force?The box is not moving in the y direction, so the normal force must be equal to the y -component of the gravitational force. Calculating the normal force is then just a matter of plugging a few numbers in for variables in order to find the y -component of the gravitational force:2. ?We know that the force pulling the box in the positive x direction has a magnitude of mg sin 30. Using Newton’s Second Law, F = ma , we just need to solve for a :3. What is the velocity of the box when it reaches the bottom of the slope?Because we’re dealing with a frictionless plane, the system is closed and we can invoke the law of conservation of mechanical energy. At the top of the inclined plane, thebox will not be moving and so it will have an initial kinetic energy of zero ().Because it is a height h above the bottom of the plane, it will have a gravitational potential energy of U = mgh. Adding kinetic and potential energy, we find that the mechanical energy of the system is:At the bottom of the slope, all the box’s potential energy will have been converted into kinetic energy. In other words, the kinetic energy, 1⁄2 mv2, of the box at the bottom of the slope is equal to the potential energy, mgh, of the box at the top of the slope. Solving for v, we get:4. What is the work done on the box by the force of gravity in bringing it to the bottom of the inclined plane?The fastest way to solve this problem is to appeal to the work-energy theorem, which tells us that the work done on an object is equal to its change in kinetic energy. At the top of the slope the box has no kinetic energy, and at the bottom of the slope its kinetic energy is equal to its potential energy at the top of the slope, mgh. So the work done on the box is:Note that the work done is independent of how steep the inclined plane is, and is only dependent on the object’s change in height when it slides down the plane.Frictionless Inclined Planes withLet’s bring together what we’ve learned about frictionless inclined planes and pulleys on tables into one exciting über-problem:Assume for this problem that —that is, mass M will pull mass m up the slope. Now let’s ask those three all-important preliminary questions:1. Ask yourself how the system will move: Because the two masses areconnected by a rope, we know that they will have the same velocity and acceleration. We also know that the tension in the rope is constant throughout its length. Because, we know that when the system is released from rest, mass M will move downward and mass m will slide up the inclined plane.•Choose a coordinate system: Do the same thing here that we did with the previous pulley-on-a-table problem. Make the x-axis parallel to the rope, with the positive x direction being up for mass M and downhill for mass m, and the negative x direction being down for mass M and uphill for mass m. Make the y-axis perpendicular to the rope, with the positive y-axis being away from the inclined plane, and the negative y-axis being toward the inclined plane.•Draw free-body diagrams: We’ve seen how to draw free-body diagrams for masses suspended from pulleys, and we’ve seen how to draw free-body diagrams for masses on inclined planes. All we need to do now is synthesize what we already know:Now let’s tackle a couple of questions:1. What is the acceleration of the masses?2. What is the velocity of mass m after mass M has fallen a distance h?1. What is the acceleration of the masses?First, let’s determine the net force acting on each of the masses. Applying Newton’s Second Law we get:Adding these two equations together, we find that . Solving for a, we get:Because , the acceleration is negative, which, as we defined it, is down for mass M and uphill for mass m.2. What is the velocity of mass m after mass M has fallen a distance h?Once again, the in-clined plane is frictionless, so we are dealing with a closed system and we can apply the law of conservation of mechanical energy. Since the masses areinitially at rest, . Since mass M falls a distance h, its potential energy changesby –-Mgh. If mass M falls a distance h, then mass m must slide the same distance up the slope of the inclined plane, or a vertical distance of . Therefore, mass m’s potential energy increases by . Because the sum of potential energy and kinetic energy cannot change, we know that the kinetic energy of the two masses increases precisely to the extent that their potential energy decreases. We have all we need to scribble out some equations and solve for v:Finally, note that the velocity of mass m is in the uphill direction.As with the complex equations we encountered with pulley systems above, you needn’t trouble yourself with memorizing a formula like this. If you understand the principles at work in this problem and would feel somewhat comfortable deriving this formula, you know more than SAT II Physics will likely ask of you.Inclined Planes With FrictionThere are two significant differences between frictionless inclined plane problems and inclined plane problems where friction is a factor:1. There’s an extra force to deal with. The force of friction will oppose the downhillcomponent of the gravitational force.2. We can no longer rely on the law of conservation of mechanical energy.Because energy is being lost through the friction between the mass and the inclined plane, we are no longer dealing with a closed system. Mechanical energy is not conserved.Consider the 10 kg box we encountered in our example of a frictionless inclined plane.This time, though, the inclined plane has a coefficient of kinetic friction of . How will this additional factor affect us? Let’s follow three familiar steps:1. Ask yourself how the system will move: If the force of gravity is strong enoughto overcome the force of friction, the box will accelerate down the plane. However,because there is a force acting against the box’s descent, we should expect it to slide witha lesser velocity than it did in the example of the frictionless plane.2. Choose a coordinate system: There’s no reason not to hold onto the co-ordinatesystem we used before: the positive x direction is down the slope, and the positive ydirection is upward, perpendicular to the slope.3. Draw free-body diagrams: The free-body diagram will be identical to the one wedrew in the example of the frictionless plane, except we will have a vector for the force of friction in the negative x direction.Now let’s ask some questions about the motion of the box.1. What is the force of kinetic friction acting on the box?2. What is the acceleration of the box?3. What is the work done on the box by the force of kinetic friction?What is the force of kinetic friction acting on the box?The normal force acting on the box is 86.6 N, exactly the same as for the frictionless inclined plane. The force of kinetic friction is defined as , so plugging in the appropriate values for and N:Remember, though, that the force of friction is exerted in the negative x direction, so the correct answer is –43.3 N.What is the acceleration of the box?The net force acting on the box is the difference between the downhillgravitational force and the force of friction: . Using Newton’s Second Law, we can determine the net force acting on the box, and then solve for a:Because , the direction of the acceleration is in the downhill direction.What is the work done on the box by the force of kinetic friction?Since W = F · d, the work done by the force of friction is the product of the force of friction and the displacement of the box in the direction that the force is exerted. Because the force of friction is exerted in the negative x direction, we need to find the displacement of the box in the x direction. We know that it has traveled a horizontal distance of d and a vertical distance of h. The Pythagorean Theorem then tells us that the displacement of thebox is . Recalling that the force of friction is –43.3 N, we know that the work done by the force of friction isNote that the amount of work done is negative, because the force of friction acts in the opposite direction of the displacement of the box.we know that when people are placed on slippery inclines, they slide down the slope. We also know that slides can sometimes be sticky, so that when you are at the top of the incline, you need to give yourself a push to overcome the force of static friction. As you descend a sticky slide, the force of kinetic friction opposes your motion. In this section, we will consider problems involving inclined planes both with and without friction. Since they’re simpler, we’ll begin with frictionless planes.Suppose you place a 10 kg box on a frictionless 30º inclined plane and release your hold, allowing the box to slide to the ground, a horizontal distance of d meters and a vertical distance of h meters.Before we continue, let’s follow those three important preliminary steps for solving problems in mechanics:1. Ask yourself how the system will move: Because this is a frictionless plane,there is nothing to stop the box from sliding down to the bottom. Experience suggests that the steeper the incline, the faster an object will slide, so we can expect the acceleration and velocity of the box to be affected by the angle of the plane.2. Choose a coordinate system: Because we’re interested in how the box slidesalong the inclined plane, we would do better to orient our coordinate system to the slope of the plane. The x-axis runs parallel to the plane, where downhill is the positive xdirection, and the y-axis runs perpendicular to the plane, where up is the positive ydirection.3. Draw free-body diagrams: The two forces acting on the box are the force ofgravity, acting straight downward, and the normal force, acting perpendicular to theinclined plane, along the y-axis. Because we’ve oriented our coordinate system to the slope of the plane, we’ll have to resolve the vector for the gravitational force, mg, into its x- and y-components. If you recall what we learned about vector decomposition inChapter 1, you’ll know you can break mg down into a vector of magnitude cos 30º in the negative y direction and a vector of magnitude sin 30º in the positive x direction. The result is a free-body diagram that looks something like this:Decomposing the mg vector gives a total of three force vectors at work in this diagram: the y-component of the gravitational force and the normal force, which cancel out; and the x-component of the gravitational force, which pulls the box down the slope.Note that the steeper the slope, the greater the force pulling the box down the slope.Now let’s solve some problems. For the purposes of these problems, take the acceleration due to gravity to be g = 10 m/s2. Like SAT II Physics, we will give you the values of the relevant trigonometric functions: cos 30 = sin 60 = 0.866, cos 60 = sin 30 =0.500.SAT物理考试-SpringsThe that springs exhibit applies equally to objects moving in a circular path and to the various wave phenomena that we’ll study later in this book. So before we dig in to the nitty-gritty of your typical SAT II Physics spring questions, let’s look at some generalfeatures of harmonic motion.and Harmonic MotionConsider the following physical phenomena:•When you drop a rock into a still pond, the rock makes a big splash, which causes ripples to spread out to the edges of the pond.•When you pluck a guitar string, the string vibrates back and forth.•When you rock a small boat, it wobbles to and fro in the water before coming to rest again.•When you stretch out a spring and release it, the spring goes back and forth between being compressed and being stretched out.There are just a few examples of the widespread phenomenon of oscillation.Oscillation is the natural world’s way of returning a system to its equilibrium position, the stable position of the system where the net force acting on it is zero. If you throw a systemoff-balance, it doesn’t simply return to the way it was; it oscillates back and forth about the equilibrium position.A system oscillates as a way of giving off energy. A system that is thrown off-kilter has more energy than a system in its equilibrium position. To take the simple example of a spring, a stretched-out spring will start to move as soon as you let go of it: that motion is evidence of kinetic energy that the spring lacks in its equilibrium position. Because of the law of conservation of energy, a stretched-out spring cannot simply return to its equilibrium position; it must release some energy in order to do so. Usually, this energy is released as thermal energy caused by friction, but there are plenty of interesting exceptions. For instance, a plucked guitar string releases sound energy: the music we hear is the result of the string returning to its equilibrium position.The movement of an oscillating body is called harmonic motion. If you were to graph the position, velocity, or acceleration of an oscillating body against time, the result would be a sinusoidal wave; that is, some variation of a y = a sin bx or a y = a cos bx graph. This generalized form of harmonic motion applies not only to springs and guitar strings, but to anything that moves in a cycle. Imagine placing a pebble on the edge of a turntable, and watching the turntable rotate while looking at it from the side. You will see the pebble moving back and forth in one dimension. The pebble will appear to oscillate just like a spring: it will appear to move fastest at the middle of its trajectory and slow to a halt and reverse direction as it reaches the edge of its trajectory.This example serves two purposes. First, it shows you that the oscillation of springs is just one of a wide range of phenomena exhibiting harmonic motion. Anything that moves in a cyclic pattern exhibits harmonic motion. This includes the light and sound waves without which we would have a lot of trouble moving about in the world. Second, we bring it up because SAT II Physics has been known to test students on the nature of the horizontal or vertical component of the motion of an object in circular motion. As you can see, circular motion viewed in one dimension is harmonic motion.Though harmonic motion is one of the most widespread and important of physical phenomena, your understanding of it will not be taxed to any great extent on SAT II Physics. In fact, beyond the motion of springs and pendulums, everything you will need toknow will be covered in this book in the chapter on Waves. The above discussion is mostly meant to fit your understanding of the oscillation of springs into a wider context.The Oscillation of a SpringNow let’s focus on the harmonic motion exhibited by a spring. To start with, we’ll imagine a mass, m, placed on a frictionless surface, and attached to a wall by a spring. In its equilibrium position, where no forces act upon it, the mass is at rest. Let’s label this equilibrium position x = 0. Intuitively, you know that if you compress or stretch out the spring it will begin to oscillate.Suppose you push the mass toward the wall, compressing the spring, until the mass isin position .When you release the mass, the spring will exert a force, pushing the mass back until itreaches position , which is called the amplitude of the spring’s motion, or the maximum displacement of the oscillator. Note that .By that point, the spring will be stretched out, and will be exerting a force to pull the mass back in toward the wall. Because we are dealing with an idealized frictionless surface, the mass will not be slowed by the force of friction, and will oscillate back andforth repeatedly between and .Hooke’s LawThis is all well and good, but we can’t get very far in sorting out the amplitude, the velocity, the energy, or anything else about the mass’s motion if we don’t understand the manner in which the spring exerts a force on the mass attached to it. The force, F, that the spring exerts on the mass is defined by Hooke’s Law:where x is the spring’s displacement from its equilibrium position and k is a constant of proportionality called the spring constant. The spring constant is a measure of “springiness”: a greater value for k signifies a “tighter” spring, one that is more resistant to being stretched.Hooke’s Law tells us that the further the spring is displaced from its equilibrium position (x) the greater the force the spring will exert in the direction of its equilibrium position (F). We call F a restoring force: it is always directed toward equilibrium. Because F and x are directly proportional, a graph of F vs. x is a line with slope –k.Simple Harmonic OscillationA mass oscillating on a spring is one example of a simple harmonic oscillator. Specifically, a simple harmonic oscillator is any object that moves about a stable equilibrium point and experiences a restoring force proportional to the oscillator’s displacement.For an oscillating spring, the restoring force, and consequently the acceleration, are greatest and positive at . These quantities decrease as x approaches the equilibrium position and are zero at x = 0. The restoring force and acceleration—which arenow negative—increase in magnitude as x approaches and are maximally negativeat .Important Properties of a Mass on a SpringThere are a number of important properties related to the motion of a mass on a spring, all of which are fair game for SAT II Physics. Remember, though: the test makers have no interest in testing your ability to recall complex formulas and perform difficult mathematical operations. You may be called upon to know the simpler of these formulas, but not the complex ones. As we mentioned at the end of the section on pulleys, it’s less important that you memorize the formulas and more important that you understand what they mean. If you understand the principle, there probably won’t be any questions that will stump you.Period of OscillationThe period of oscillation, T, of a spring is the amount of time it takes for a spring to complete a round-trip or cycle. Mathematically, the period of oscillation of a simple harmonic oscillator described by Hooke’s Law is:This equation tells us that as the mass of the block, m, increases and the spring constant, k, decreases, the period increases. In other words, a heavy mass attached to an easily stretched spring will oscillate back and forth very slowly, while a light mass attached to a resistant spring will oscillate back and forth very quickly.FrequencyThe frequency of the spring’s motion tells us how quickly the object is oscillating, or how many cycles it completes in a given timeframe. Frequency is inversely proportional to period:Frequency is given in units of cycles per second, or hertz (Hz).Potential EnergyThe potential energy of a spring () is sometimes called elastic energy, because itresults from the spring being stretched or compressed. Mathematically, is defined by:The potential energy of a spring is greatest when the coil is maximally compressed or stretched, and is zero at the equilibrium position.Kinetic EnergySAT II Physics will not test you on the motion of springs involving friction, so for the purposes of the test, the mechanical energy of a spring is a conserved quantity. As we recall, mechanical energy is the sum of the kinetic energy and potential energy.At the points of maximum compression and extension, the velocity, and hence the kinetic energy, is zero and the mechanical energy is equal to the potential energy, U s=1/.2At the equilibrium position, the potential energy is zero, and the velocity and kinetic energy are maximized. The kinetic energy at the equilibrium position is equal to the mechanical energy:From this equation, we can derive the maximum velocity:You won’t need to know this equation, but it might be valuable to note that the velocity increases with a large displacement, a resistant spring, and a small mass.SummaryIt is highly unlikely that the formulas discussed above will appear on SAT II Physics. More likely, you will be asked conceptual questions such as: at what point in a spring’s oscillation is the kinetic or potential energy maximized or minimized, for instance. The figure below summarizes and clarifies some qualitative aspects of simple harmonic oscillation. Your qualitative understanding of the relationship between force, velocity, and kinetic and potential energy in a spring system is far more likely to be tested than your knowledge of the formulas discussed above.In this figure, v represents velocity, F represents force, KE represents kinetic energy, and represents potential energy.Vertical Oscillation of SpringsNow let’s consider a mass attached to a spring that is suspended from the ceiling. Questions of this sort have a nasty habit of coming up on SAT II Physics. The oscillation ofthe spring when compressed or extended won’t be any different, but we now have to take gravity into account.Equilibrium PositionBecause the mass will exert a gravitational force to stretch the spring downward a bit, the equilibrium position will no longer be at x = 0, but at x = –h, where h is the vertical displacement of the spring due to the gravitational pull exerted on the mass. The equilibrium position is the point where the net force acting on the mass is zero; in other words, the point where the upward restoring force of the spring is equal to the downward gravitational force of the mass.Combining the restoring force, F = –kh, and the gravitational force, F = mg, we can solve for h:Since m is in the numerator and k in the denominator of the fraction, the mass displaces itself more if it has a large weight and is suspended from a lax spring, as intuition suggests.A Vertical Spring in MotionIf the spring is then stretched a distance d, where d < h, it will oscillate between and .Throughout the motion of the mass, the force of gravity is constant and downward. The restoring force of the spring is always upward, because even atthe mass is below the spring’s initial equilibrium position of x = 0. Note that if dwere greater than h, would be above x = 0, and the restoring force would act in the downward direction until the mass descended once more below x = 0.According to Hooke’s Law, the restoring force decreases in magnitude as the spring is compressed. Consequently, the net force downward is greatest at and the netforce upward is greatest at .EnergyThe mechanical energy of the vertically oscillating spring is:where is gravitational potential energy and is the spring’s (elastic) potential energy.Note that the velocity of the block is zero at and , and maximized at the equilibrium position, x = –h. Consequently, the kinetic energy of the spring is zero for and and is greatest at x = –h. The gravitational potential energy of the system increases with the height of the mass. The elastic potential energy of the spring isgreatest when the spring is maximally extended at and decreases with the extension of the spring.How This Knowledge Will Be TestedMost of the questions on SAT II Physics that deal with spring motion will ask qualitatively about the energy or velocity of a vertically oscillating spring. For instance, you may be shown a diagram capturing one moment in a spring’s trajectory and asked about the relative magnitudes of the gravitational and elastic potential energies and kineticenergy. Or you may be asked at what point in a spring’s trajectory the velocity ismaximized. The answer, of course, is that it is maximized at the equilibrium position. It is far less likely that you will be asked a question that involves any sort of calculation.The that springs exhibit applies equally to objects moving in a circular path and to the various wave phenomena that we’ll study later in this book. So before we dig in to the nitty-gritty of your typical SAT II Physics spring questions, let’s look at some generalfeatures of harmonic motion.and Harmonic MotionConsider the following physical phenomena:•When you drop a rock into a still pond, the rock makes a big splash, which causes ripples to spread out to the edges of the pond.•When you pluck a guitar string, the string vibrates back and forth.•When you rock a small boat, it wobbles to and fro in the water before coming to rest again.•When you stretch out a spring and release it, the spring goes back and forth between being compressed and being stretched out.There are just a few examples of the widespread phenomenon of oscillation.Oscillation is the natural world’s way of returning a system to its equilibrium position, the stable position of the system where the net force acting on it is zero. If you throw a system off-balance, it doesn’t simply return to the way it was; it oscillates back and forth about the equilibrium position.A system oscillates as a way of giving off energy. A system that is thrown off-kilterhas more energy than a system in its equilibrium position. To take the simple example of a spring, a stretched-out spring will start to move as soon as you let go of it: that motion is evidence of kinetic energy that the spring lacks in its equilibrium position. Because of the law of conservation of energy, a stretched-out spring cannot simply return to itsequilibrium position; it must release some energy in order to do so. Usually, this energy is released as thermal energy caused by friction, but there are plenty of interesting。
