WENO Schemes for Cylindrical and Spherical Grid
Synthesis and characterization of novel systems fo

专利内容由知识产权出版社提供
专利名称:Synthesis and characterization of novel systems for guidance and vectorization of molecules of therapeutic interest towards target cells
发明人:Pascal Dumy,Marie-Christine Favrot,Didier Boturyn,Jean-Luc Coll
申请号:US10528320 申请日:20030919 公开号:US07531622B2 公开日:20090512
摘要:A method for preparing a grafted homodetic cyclopeptide forming a framework that defines a grafted upper face and grafted lower face, including synthesizing a linear peptide from modified or unmodified amino acids, some of which carry orthogonal protective groups; intramolecular cyclizing the resulting protetuting some or all of orthogonal protective groups with a protected precursor; and grafting at least one molecule of interest onto one and/or the other face of the framework via an oxime bond.
5.翻译原文

关于绍兴的科学家作文英语

关于绍兴的科学家作文英语Shaoxing, a city steeped in history and culture, has been home to numerous scientists who have made significant contributions to the field of science and technology. This essay aims to highlight the achievements of some of these remarkable individuals and the impact they have had on the world.The city's rich intellectual heritage is evident in the lives of its scientists. One such luminary is Mr. Wu, a renowned physicist who has dedicated his life to the study of quantum mechanics. His research has shed light on the fundamental principles governing the universe, earning him international acclaim and several prestigious awards.Another notable figure is Dr. Li, a biotechnologist who has made groundbreaking advancements in genetic engineering. Her work has revolutionized the way we understand and manipulate genetic material, leading to new treatments for various diseases and a deeper understanding of the human genome.The contributions of these scientists extend beyond the realm of academia. Ms. Chen, an environmental scientist, has been at the forefront of sustainable development initiatives in Shaoxing. Her efforts have not only improved the quality of life for the city's residents but also set a standard foreco-friendly practices that other cities aspire to follow.Moreover, the spirit of innovation is alive and well among the younger generation of scientists in Shaoxing. Young researchers like Mr. Zhang are already making waves in their respective fields.张先生, a prodigious chemist, has developed novel materials with applications in energy storage and electronics, showcasing the potential of Shaoxing'sscientific community to innovate and adapt to the challenges of the future.In conclusion, the scientists of Shaoxing are a testament to the city's commitment to scientific excellence and progress. Their work has not only enriched the scientific community but also improved the lives of people around the world. As we celebrate their achievements, we are reminded of the importance of fostering a culture of curiosity, inquiry, and innovation that has been the hallmark of Shaoxing'sscientific legacy.This essay is a tribute to the scientists of Shaoxing, whose tireless pursuit of knowledge continues to inspire and shape the world we live in.。
翻译

在本文中,我们通过GGA-PBE(广义梯度-pridew-burke-ernzerhof)近似的应用密度泛函理论研究了锯齿型石墨烯纳米带应力的存在的结构,电子和机械性能。
单向应力沿周期方向施加,从而引起在±0.02%范围内的单一的变形。
这样的机械性能表明该范围内是线性响应,而非线性的响应依赖于更高的应变。
最相关的结果表明,它的杨氏模量比已经确定的石墨和碳纳米管的更高。
C-C键在边缘的几何重建使纳米结构变得坚固。
这种电子结构的特点是不对这个线弹性范围的应变敏感,表明了碳纳米结构在不久的将来在纳米电子器件中使用的的可能性。
引言最近,一个新的碳纳米结构,被称为石墨烯纳米带(GNR)已经出现,并且因为承诺能在自旋电子学中使用引起了科学界的关注。
这主要是归功于son 等人的研究,他预测面内电场垂直于周期性轴时,诱导半金属态的锯齿形纳米带(ZGNR)。
除了这种电子结构对电场的有趣的依赖性,这对于将来的自旋设备是一种很有前途的材料,因为它可以作为一个完美的自旋过滤器。
最近坎波斯 - 德尔加多等人为大量生产长而薄,且高度结晶的石墨带(小于20-30微米的长度),宽度为20〜300nm且厚度小(2-40层)。
报道了一种化学气相沉积路线(CVD)。
本实验预先进一步增加了在高科技设备中使用这些材料的期望。
同时,由于其出色的机械和电子特性,需要增加碳纳米结构的一般物理特性的兴趣。
最近, Lee等人通过测定单一的石墨烯层的机械性能,表明了石墨烯是已知的最坚固的材料,因为它的弹性模量达到了1.0 TPA。
此外,更多的努力一直致力于研究石墨烯的电子特性,因为创造一个缺口就可能允许在场效应晶体管中使用石墨烯。
许多机制已经被提出来用于这一目的:纳米图案化,形成量子点,采用多层,共价功能化,掺杂如硫一样的杂原子和施加机械应力。
在最后这种情况下,线弹性理论中和紧束缚方法,佩雷拉等人发现,应变能产生大量的频谱差距。
但是这种差距是关键的,需要超过临界值20%,并且仅沿相对于所述底层优选的方向。
Howlongandinwhatcapacityhaveyouknowntheapplicant

To Whom It May Concern:Recommendation Letter for Marcus Kee Woon TanMy replies:a. How long and in what capacity have you known the applicant?Marcus is my cousin and we have maintained regular contact.b. How does the applicant stand out from others in a similar capacity?Marcus is brilliant, articulate, and a born leader. I put him under “star” category for his ability to solve problems and to inspire others. I rate him as one of the top three young adults I know in his cohort.c. Please provide an example of the applicant’s impact on a person, group or organization.In NUS, Marcus was placed in the Accelerated Masters Programme, a highly selective program that is only offered to the most outstanding undergraduate students. Marcus produced a thesis that was truly first-rate. His research in microwave technology involved complex mathematical analysis of novel microwave transmission lines. Using the conformal mapping technique, he was able to derive simple mathematical equations of key characteristics of the asymmetric coplanar waveguide. Previously, the asymmetric coplanar waveguide can only be analysed by using long polynomial equations (developed by Prof Yamashita) that have to be solved by a computer.Marcus’s research had s ignificant application in real-world microwave circuit analysis. In December 1998, he was the only Singapore research student to do an oral presentation of his Master research work in Asia Pacific Microwave Conference (APMC) 98, Yokohama, Japan. Subsequently, his Master research paper was accepted for publication by IEICE Transactions.d. Please provide a representative example of how the applicant interacts with other people.Marcus has the rare gift of inspiring others. He is a team-player and an asset in any group task. He knows how to “get” people going without making them feel “pushed”.e. Which of the applicant’s personal or professional characteristics would you change?I see his major weakness as lack of patience. When Marcus was younger, his impatience could come across as arrogance. Marcus was aware of this problem and has been making good progress towards correcting this. He is now very sensitive towards others’ needs.f. Please tell us anything else you think we should know about this applicant. Marcus wants to be a high-tech entrepreneur. This purpose fuels his drive, passion, and motivation for work. He is so well-read in high-tech businesses that I sometimes consult him about happenings in the industry (when preparing for my MBA classes). I believe his determination will make his dream come true.Marcus is a great leader and communicator. I have no doubt he will assume a senior management position in the years to come.Yours truly,Teck-Hua HoWilliam Halford, Jr. Family Professor of MarketingUniversity of California, Berkeley, Haas School of Business。
作者姓名:卢滇楠

附件6作者姓名:卢滇楠论文题目:温敏型高分子辅助蛋白质体外折叠的实验和分子模拟研究作者简介:卢滇楠,男,1978年4月出生, 2000年9月师从清华大学化工系生物化工研究所刘铮教授,从事蛋白质体外折叠的分子模拟和实验研究,于2006年1月获博士学位。
博士论文成果以系列论文形式集中发表在相关研究领域的权威刊物上。
截至2007年发表与博士论文相关学术论文21篇,其中第一作者SCI论文9篇(有4篇IF>3),累计他引20次(SCI检索),EI收录论文14篇(含双收),国内专利1项。
中文摘要引言蛋白质体外折叠是重组蛋白质药物生产的关键技术,也是现代生物化工学科的前沿领域之一,大肠杆菌是重要的重组蛋白质宿主体系,截止2005年FDA批准的64种重组蛋白药物中有26种采用大肠杆菌作为宿主体系,目前正在研发中的4000多种蛋白质药物中有90%采用大肠杆菌为宿主表达体系。
但由于大肠杆菌表达系统缺乏后修饰体系使得其生产的目标蛋白质多以无生物学活性的聚集体——包涵体的形式存在,在后续生产过程中需要对其进行溶解,此时蛋白质呈无规伸展链状结构,然后通过调整溶液组成诱导蛋白质发生折叠形成具有预期生物学活性的高级结构,这个过程就称之为蛋白质折叠或者复性,由于该过程是在细胞外进行的,又称之为蛋白质体外折叠技术。
蛋白质体外折叠技术要解决的关键问题是避免蛋白质的错误折叠以及形成蛋白质聚集体。
目前本领域的研究以具体技术和产品折叠工艺居多,折叠过程研究方面则多依赖宏观的结构和性质分析如各类光谱学和生物活性测定等,在研究方法上存在折叠理论、分子模拟与实验研究结合不够的问题,这些都不利于折叠技术的发展和应用。
本研究以发展蛋白质新型体外折叠技术为目标,借鉴蛋白质体内折叠的分子伴侣机制,提出以智能高分子作为人工分子伴侣促进蛋白质折叠的新思路,即通过调控高分子与蛋白质分子的相互作用,1)诱导伸展态的变性蛋白质塌缩形成疏水核心以抑制蛋白质分子间疏水作用所导致的聚集,2)与折叠中间态形成多种可逆解离复合物,丰富蛋白质折叠的途径以提高折叠收率。
论文翻译

当III族氮化物进入红外波段:新的性能和前景宽禁带半导体材料GaN和富镓组分的InGaN合金,其能带覆盖范围从蓝光到近紫外波段,是在固态照明和激光技术领域拥有很大优势的材料,因此,已很好地得到研究。
然而一直致力于InN和富铟组分的InGaN合金的努力都事半功倍,直到2002年的一个重大突破-铟的禁带宽度从1.9 eV减少至0.64 eV,这一突破源自于分子束外延技术大大提高了InN薄膜生长的质量。
这一发现使得窄禁带III族氮化物得到广泛的研究。
InN较低的禁带宽度为含有各种成分的InGaN 和InAlN合金的电子结构的描述提供了基础。
它在更宽的光谱区扩展了III族氮化物合金系的基本带宽,从1.9微米的近红外(铟的禁带宽度为0.64eV)到0.36微米(氮化镓的禁带宽度为3.4 eV)或者0.2微米的紫外线(氮化铝的禁带宽度为6.2eV)。
能带的连续范围现在已经扩展到近红外,提高了III族氮化物新的应用的可能性。
在这篇文章中,我们对InN和相关III族氮化物半导体的物理性能进行了详细审查。
在对InN能带的重新评估中我们将讨论电子结构,载流子动力学,光子跃迁,物理缺陷,掺杂的不同,表面效应以及声子结构。
然后,我们将描述基于InGaN合金新的电子器件和光电子器件发展的进展,前景以及所面临的挑战。
我们将对InN和InGaN的纳米结构和相应的InN和InGaN薄膜的表征和了解都进行审查。
第一章引言光电市场目前的规模达到200亿美元每年,预计很快在未来几十年内进一步扩大。
这很大程度上是由于经济增长和固态照明以及激光技术的提高。
20世纪90年代出现的铟镓氮作为有源层的高亮度的蓝光发光二极管促进了这一增长。
因此,在这一领域中,大量的研究集中在富镓组分的InGaN和GaAlN合金,其能带隙覆盖范围从蓝光到近紫外波段。
从那时起,迅速发展的固态照明技术给光电字学及光学领域带来了革命。
从图中可以看出,InGaN在该领域起主导作用,其带宽覆盖范围从蓝光到绿光(相应的波长为400到530nm或光子能量从3.1eV 到2.3 eV)。
Biosensors and Bioelectronics

Biosensors and Bioelectronics Biosensors and bioelectronics have revolutionized the field of healthcare and biotechnology, offering innovative solutions for disease diagnosis, environmental monitoring, and biometric applications. These cutting-edge technologies have the potential to improve the quality of life for individuals worldwide, making significant strides in the detection and analysis of biological substances. The integration of biosensors and bioelectronics has paved the way for more efficient and accurate medical diagnostics, environmental monitoring, and food safety testing, among other applications. This article will explore the multifaceted impact of biosensors and bioelectronics from various perspectives, highlighting their significance in modern society. From a healthcare perspective, biosensors and bioelectronics have transformed the landscape of medical diagnostics andpatient care. These advanced technologies enable the rapid and precise detectionof biomarkers, pathogens, and other biological substances, leading to earlydisease diagnosis and personalized treatment strategies. For instance, biosensors can detect specific cancer biomarkers in blood samples, allowing for early intervention and improved patient outcomes. Furthermore, the development of wearable biosensors has empowered individuals to monitor their health in real time, providing valuable insights for preventive healthcare and chronic disease management. The integration of bioelectronics in medical devices has also enhanced the delivery of therapeutic treatments, such as targeted drug delivery systems and implantable bioelectronic devices for neurological disorders. Overall, biosensors and bioelectronics have significantly contributed to the advancement of modern medicine, offering new possibilities for disease management and personalized healthcare. In the realm of environmental monitoring, biosensors andbioelectronics play a pivotal role in assessing and mitigating environmental risks. These technologies enable the detection of pollutants, toxins, and microbial contaminants in air, water, and soil, thereby supporting environmentalconservation and public health protection. For example, biosensors can be deployed for real-time monitoring of water quality in natural ecosystems or industrial settings, providing early warnings of potential contamination events. Additionally, bioelectronic devices equipped with environmental sensors can contribute to thesurveillance of air pollution levels and the impact of urban development on ecological systems. By harnessing the power of biosensors and bioelectronics, environmental scientists and policymakers can make informed decisions to safeguard natural resources and mitigate the impact of human activities on the environment. In the context of food safety and agriculture, biosensors and bioelectronics offer innovative solutions for quality control and production efficiency. These technologies enable the rapid detection of foodborne pathogens, allergens, and contaminants, ensuring the safety and integrity of the food supply chain. By integrating biosensors into food processing facilities and agricultural practices, potential hazards can be identified and addressed proactively, reducing the riskof foodborne illnesses and product recalls. Moreover, bioelectronic devices can facilitate precision agriculture by monitoring soil conditions, crop health, and environmental factors, optimizing resource utilization and maximizing crop yields. The application of biosensors and bioelectronics in the food and agricultureindustry contributes to sustainable practices, consumer protection, and globalfood security, addressing critical challenges in a rapidly evolving world. From a technological and scientific perspective, the development of biosensors and bioelectronics continues to drive innovation and interdisciplinary collaboration. Researchers and engineers are constantly pushing the boundaries of sensor design, material science, and bioelectronic interfaces to enhance sensitivity, selectivity, and usability. The integration of nanotechnology, microfluidics, andbioinformatics has expanded the capabilities of biosensors, enabling the detection of complex biological interactions with unprecedented precision. Furthermore, the convergence of bioelectronics with artificial intelligence and machine learninghas opened new frontiers in data analysis, signal processing, and diagnostic interpretation, fostering the development of smart, adaptive biosensing platforms. As a result, biosensors and bioelectronics are at the forefront of scientific exploration, offering a rich landscape for discovery and technological advancement. In conclusion, biosensors and bioelectronics have emerged as transformative tools with far-reaching implications for healthcare, environmental monitoring, food safety, and scientific innovation. These technologies have redefined the landscape of diagnostics, monitoring, and analysis, empowering individuals, industries, andsocieties to make informed decisions and improve quality of life. As we look to the future, the continued advancement of biosensors and bioelectronics holds promise for addressing global health challenges, environmental sustainability, and technological progress. By embracing the potential of biosensors and bioelectronics, we can unlock new opportunities for societal benefit and create a healthier, more connected world.。
1982年诺贝尔生理学医学奖

—关于前列腺素和有关活性物质的发现
The Nobel Prize in Physiology or Medicine 1982
"for their discoveries concerning prostaglandins and related biologically active substances"
Sune K. Bergström – Autobiography
I was born January 10th, 1916 in Stockholm, Sweden. 1944 1946 - 47 1947 - 58 1958 - 80 1963 - 66 D. Med. Sci., Biochemistry, Karolinska Institutet, Stockholm Research Fellowship, Basel University, Basel Professor of Physiological Chemistry, The University of Lund, Lund Professor of Chemistry, Karolinska Institutet, Stockholm Dean of the Medical Faculty, Karolinska Institutet, Stockholm Rector of Karolinska Institutet, Stockholm Chairman of the Board of Directors, The Nobel Foundation, Stockholm Nobel Laureate in Physiology or Medicine,Stockholm President of the Royal Swedish Academy of Sciences
Modeling, analysis and simulation

The research in MAS concentrates on the qualitative, numerical and computational aspects of mathema-tical models arising in a wide range of applications within the Dutch society.Particular attention is gi-ven to models describing continuum processes.As examples we mention freefluid and porous mediaflow,chemical reactions in the atmosphere and cir-cuit analysis.The applications are combined into two extensive programmes or themes,each contai-ning a number of characteristic projects.They are accounted for in detail in the theme descriptions of MAS1and MAS2.New developments include the projects MAS2.1(Computationalfluid dynamics,a collaboration with MARIN)and MAS2.7(Mathe-matics of Finance).Preliminary contacts have been made with TNO-TPD and across the border with GMD in Germany.It is expected that new projects will emerge from these contacts.In the midst of much applied work,MAS conti-nues to contribute significantly to the scientific de-velopment in the areas of qualitative,numerical and computational methods for partial differential equati-ons.The number of papers appeared in international journals is certainly satisfactory,and withfive PhD theses MAS contributes substantially to the scientific output of the CWI.Separately from the themes,the Dynamical Sys-tems Laboratory(DSL)operated as an independent unit.During the past years DSL has played a leading role in the software development for bifurcation of equilibria of systems of ordinary differential equa-tions.An extensive description of their activities is given in the DSL contribution.This year was the last year of DSL,which officially ended when Dr.Yu.A. Kuznetsov left the CWI(November1st,1997). MAS is very pleased to have Professor Piet van der Houwen as a CWI Fellow in its ranks.His high level scientific input,interaction with young re-searchers and involvement with several project is very much appreciated.Dynamical Systems Laboratory–DSL-Yu.A.Kuznetsov-V.V.Levitin -J.A.SandersEnvironmental Modelling and Porous Media Re-search–MAS1-J.G.Verwer-C.J.van Duijn-J.Hulshof-P.J.van der Houwen-J.G.Blom-C.Cuesta-M.I.J.van Dijke-G.Galiano-W.Hundsdorfer-J.Koknserstdrager-M.A.A.van Leeuwen-W.M.Lioen-M.van Loon-J.Molenaar-R.J.Schotting-B.P.Sommeijer-E.J.Spee-J.de Vries-P.M.de ZeeuwIndustrial Processes–MAS2-E.H.van Brummelen-S.Cavallar-M.K.C¸amlıbel-M.Genseberger-T.Hantke-P.W.Hemker-J.Hoogland-P.J.van der Houwen-K.Karamazen-M.Kirkilionis-A.de Koeijer-B.Korennserstdrager-W.M.Lioen-P.L.Montgomery-M.Nool-J.Noordmans-O.Penninga-A.van der Ploeg-H.J.J.te Riele-A.J.van der Schaft-J.M.Schumacher-B.P.Sommeijer-W.J.H.Stortelder-J.B.de Swart-N.M.Temme-W.A.van der Veen-D.T.Winter-P.WesselingSecretary:N.MitrovicJ.A.SandersYu.A.KuznetsovV.V.LevitinDuring1997,two versions of CONTENT,1.3and1.4,were released on ftp.cwi.nl in directorypub/CONTENT.Release1.4–December,1997New features:-new class of dynamical systems is supported: differential-algebraic equations(DAEs)Mx’=f(x,p) with possibly singular matrix M;-numerical integration of stiff ODEs and DAEs using RADAU5code;-three-parameter continuation of all codim2bifur-cations of ODEs(cusp,Bogdanov-Takens,genera-lized Hopf,zero-Hopf,and double Hopf);-two-parameter continuation of Hopf bifurcation using the bordered squared Jacobian matrix;-symbolical calculation of derivatives of the4th or-der using the Maple system.Release1.3–August,1997New features:-one-parameter continuation of limit cycles in ODEs;-detection and branch switching at codim1bifurca-tions of limit cycles;-one-parameter continuation offixed points of ite-rated maps;-detection and normal form analysis of codim1bi-furcations of iterated maps;-3D graphic windows;-Staircase window for scalar iterated maps.At the moment,CONTENT completely supportsone-parameter analysis of equilibria and cycles in ODEs and iterated maps.To complete the support of two-parameter analysis of ODEs,one has to imple-ment the continuation of codim1bifurcations of cy-cles(i.e.,fold,period-doubling,and Neimark-Sacker bifurcations),as well as the homoclinic bifurcati-ons of saddle and saddle-node equilibria,and branch switching between them.CONTENT provides the environment to implement all of these continuations. Also,the computation of the remaining normal form coefficients at codim2bifurcations of equilibria has to be implemented in CONTENT(see below).Yu.A.Kuznetsov derived explicit normal form coefficients for the reduced to the central manifold equations for all codim2equilibrium bifurcation of ODEs.A CWI Report is published.Yu.A.Kuznetsov(together with aerts and B. Sijnave,Gent University)developed new algorithms to continue codim1and2bifurcations of equilibria in2and3parameters,respectively.The algorithms were implemented in CONTENT.Yu.A.Kuznetsov(together with A.Champneys (Bristol)and B.Sandstede(Berlin))implemented the homoclinic continuation into AUTO97,the latest version of the continuation/bifurcation software by E.Doedel(Concordia University,Montreal).Now it is the standard part of AUTO.The text of the second edition of the book by Yu.A. Kuznetsov Elements of Applied Bifurcation The-ory has been sent to the Production Departmentof Springer-Verlag in September1997and will be published in1998.E.Doedel(Concordia University,Montreal,Ca-nada)June15–28.The visit was devoted to discussions of new me-thods to continue codim1bifurcations of limit cy-cles.In particular,a new method to continue the period-doubling bifurcation was proposed that combines orthogonal collocation technique with matrix bordering.O.De Feo(Swiss Federal Institute of Technology, Lausanne,Switzerland)September1–30.During the visit,RADAU5method for the numeri-cal integration of ODEs and DAEs was translated from FORTRAN to C and implemented into CON-TENT.A paper on homoclinic bifurcations in a3D food chain model was practicallyfinished.aerts and B.Sijnave(University of Gent, Belgium)June19–22.Bordering methods to continue codim1and2bi-furcation of equilibria were discussed.A proto-type method to continue the Bogdanov-Takens bi-furcation was implemented during the visit.The continuation of all other codim2bifurcations of equilibria in three parameters was implemented la-ter in1997.A.Shilnikov(Institute of Applied Mathematics and Cybernetics,Nizhnii Novgorod,Russia)May30.A lecture was given at CWI on‘A blue-sky cata-strophe model’,demonstrating a new way of a de-struction of a limit cycle.Yu.A.Kuznetsov gave invited lectures on CON-TENT at the International Workshop‘Numerical analysis of Dynamical Systems’,IMA,Minneapo-lis,USA,September15–19,and at the Workshop ‘Hybrid-methods for Bifurcation and Dynamics of Partial Differential Equations’,University of Mar-burg,June8–11.MAS-R9730.Y U.A.K UZNETSOV.Explicit nor-mal form coefficients for all codim2bifurcations of equilibria in ODEs.E.J.D OEDEL,A.R.C HAMPNEYS,T.F.F AIR-GRIEVE,Y U.A.K UZNETSOV,B.S ANDSTEDE,X.-J.W ANG(1997).AUTO97:Continuation and Bifurcation Software for Ordinary Differential Equa-tions(with HomCont).User’s Guide,Concordia University,Montreal,Canada.W.G OVAERTS,Y U.A.K UZNETSOV,B.S IJNAVE (1997).Implementation of Hopf and double Hopf Continuation Using Bordering Methods,Department of Applied Mathematics and Computer Science,Uni-versity of Ghent,Belgium.W.G OVAERTS,Y U.A.K UZNETSOV,B.S IJNAVE (1997).Computation and Continuation of Codimen-sion2Bifurcations in CONTENT,Department of Ap-plied Mathematics and Computer Science,University of Ghent,Belgium.Y U.A.K UZNETSOV(1997).Centre manifold; Codimension-two bifurcations;Equivalence of dyna-mical systems;Homoclinic bifurcations;Hopf bifur-cation;Saddle-node bifurcation.M.H AZEWINKEL (ed.).Encyclopaedia of Mathematics.Supplement Volume I,Kluwer Academic Publishers,The Nether-lands,179–181,190–101,240,293–294,296–297, 444–445.Dr.J.G.Verwer,researcher,theme leader Prof.dr.ir.C.J.van Duijn,researcher,cluster leaderDr.J.Hulshof,advisorProf.dr.P.J.van der Houwen,researcher,CWI fellowDrs.J.G.Blom,researcherMrs.C.Cuesta,Ph.D.studentDrs.M.I.J.van Dijke,Ph.D.studentDr.G.Galiano,postdocDr.W.Hundsdorfer,researcherDrs.J.Kok,researchernser,Ph.D.studentstdrager,Ph.D.studentDr.M.A.A.van Leeuwen,postdocDrs.W.M.Lioen,programmerDr.M.van Loon,postdocDr.J.Molenaar,postdocIr.R.J.Schotting,researcherDr.B.P.Sommeijer,researcherDrs.E.J.Spee,Ph.D.studentDr.J.de Vries,researcherDrs.P.M.de Zeeuw,programmer,till February1The general purpose of this research theme is to develop,analyze and implement mathematical and numerical models for application to complex pro-blems arising in environmental modelling and porous media research.MAS1is particular concerned with ordinary and partial differential equations,descri-bingfluidflow,transport of pollutants and chemical and bio-chemical processes.These differential equa-tions lie at the heart of simulation models used in atmospheric air quality modelling,in surface wa-ter and groundwater water quality modelling,and in porous media research directed for example at en-hanced oil recovery.The research subthemes cover a wide range of scientific activities,ranging from fun-damental mathematical and numerical analysis of differential equations and development of new com-putational techniques for use on vector/parallel and massively parallel computers and heterogeneous net-works(HPCN),to implementation of fully integrated models and application to real life problems.Exten-sive co-operations and contacts are maintained with researchers from the academic world and from the environmental and porous media applicationfields. Externalfinancing comes from a variety of sources, such as industry,special programs from the Nether-lands Organization for Scientific Research,research programs from the European Union and the national HPCN program funded through the Ministry of Eco-nomic Affairs.In1997research was organized in four subthemes:The research concerns the numerical modelling of the long range transport and chemical exchange of atmospheric air pollutants.Within the Netherlandsco-operation has existed with KEMA,NLR,RIVM, TUD,TNO and UU/IMAU.At the international le-vel,two joint papers with CGRER(Center for Global and Regional Environmental Research,Universityof Iowa),have been published in Atmospheric Envi-ronment(See Sandu et al.).The CWI group is also active within the European network GLOREAM and among others involved in the organization of an In-ternational Conference on Air Pollution in Paris in 1998.January23,1998,Edwin Spee will defend his Ph.D.Thesis Numerical Methods in Global Trans-port Models at the University of Amsterdam.Two new Ph.D.students have recently joined the group, viz.Debby Lanser and Boris Lastdrager.In1997 MAS1worked on the following projects:RIFTOZ–The technique of data-assimilation has been examined for improving results of model simulations by usage of actual measurements.A special implementation of an extended Kalmanfilter has been shown promising,see Report MAS-R9702 for details.The project forms part of an EU project in which CWI has been active through a subcon-tract with TUD.At CWI the project has now been terminated with the departure of Dr.M.van Loonto TNO.The Kalmanfilter will be further tested by TNO for use in their dispersion model LOTOS. LOTOS–Here the objective is to develop a regio-nal,three-dimensional,long term ozone simulation model.This LOTOS model should replace at due time an existing regional forecasting model in use at TNO.The model is developed in co-operation with TNO researchers.At TNO the focus lies on physi-cal,meteorological and chemical aspects.The CWI research focuses on the design of the mathematical model for a so-called hybrid(terrain following and pressure based)coordinate system and,in particular, of tailored numerical algorithms and implementa-tions on super and parallel computers.The project is part of the TASC project‘HPCN for Environ-mental Applications’which is funded by the Dutch HPCN program.At the end of1997the project was halfway.Afirst running operational prototype im-plemented at CWI has recently been transferred to TNO.Research details are found in the reports MAS-N9701,R9717.NCF–This one-year project is linked with the LOTOS project and concerns aspects of massive parallelism,in particular for T3E implementations. Special attention has been given to the question to which extent massive(meteo)I/O can degrade the parallel performance of models used in atmospheric simulations.Results will be reported early1998. Support is provided by the NCF/Cray University Grant program.The project lasts until April next year.Early1997the Report MAS-R9702wasfinish-ed.This publication concerns research in a similar NCF project terminated in February,1997.CIRK–This Ph.D.project has been terminatedat the end of1997with the departure of Drs.Edwin Spee.He will defend his Thesis at the Universityof Amsterdam on January23,1998.The project is similar to the LOTOS project,but here the particu-lar objective was to develop numerical algorithms for use in3D models for the whole of the global troposphere/stratosphere.In this last year we have worked on various aspects of a Rosenbrock method (see Report MAS-R9717),including stiff chemis-try integration and a factorization approach within the Rosenbrock framework.The factorization idea was investigated to provide an alternative for time or operator splitting.A second main activity has been the validation of various advection schemes in a real life radon experiment,using analyzed windfields from the ECMWF(see Report MAS-R9710).Sup-port for this project was obtained from the RIVM and very fruitful scientific co-operation has existed with IMAU/UU.This co-operation will continue in a following project,planned for the next three years. The new project is centered around the existing mo-del TM3.With support from SWON two postdocs will be hired for algorithmic and parallel software research.GOA–This activity concerns a new Ph.D.project on the‘Analysis and Validation of Operator Split-ting in Air Quality Modeling’.This project has been granted by GOA,the Netherlands Geoscien-ces Foundation.It started September1,1997with the employment of Ir.Debby Lanser.Afirst article on the analysis of Strang-splitting for PDEs of the advection-diffusion-reaction type is already in prepa-ration.SWON–A second new project Ph.D.project started December1,1997with the employment of Drs.Boris Lastdrager.This project has been granted by SWON and concerns‘Sparse Grid Methods for Time-Dependent PDEs’.Atmospheric transport-chemistry problems provide a highly useful applica-tion for sparse-grid research.The project is a joint activity between MAS1and MAS2(Dr.ir.B.Ko-ren).The research concentrates on the design of parallel numerical methods for the simulation of water pol-lution(calamitous releases),the marine eco-system,dispersion of river water,sediment transport,etc. Our activities in1997included:HPCN–In1996we started the development of a special purpose3D transport model based onfinite difference space-discretization and unconditionally stable,implicit time-discretization.In1997we ana-lyzed an iterative approach for solving the implicit relations.This iteration process is based on approxi-mate factorization such that only one-dimensionally implicit,linear systems occur in the algorithm.Inco-operation with C.Eichler-Liebenow from the University of Halle,the convergence region of the iteration method and its effect on the overall stabi-lity of the integration method has been analyzed, see Report MAS-R9718.Furthermore,we started the development of tools for domain decomposition with domains of varying grid resolutions.Part of the research was carried out within the research con-sortium TASC,with support from the Dutch HPCN programme.SWEM–The velocityfield needed by transport models either is read from inputfiles or is computed simultaneously with the computation of the pollu-tant concentrations by means of a hydrodynamical model.In view of the complicated data structures in-volved,we decided to focus on the second approach, because the hydrodynamical model can be designed such that it uses the same data structures as the trans-port model.Such an approach is justified,because the underlying partial differential equations are to a large extent identical.By choosing the same type of spatial and temporal discretizations,the same decom-position in domains with the same resolutions,and the same stepsizes in both algorithms,we achieve that the data structures are exactly the same.Since the transport solver is designed and tuned with paral-lel computer systems in mind,the velocityfield sol-ver will also be tuned to parallel computer systems. Moreover,each velocity-field-solver step can be per-formed in parallel with the corresponding transport-solver step.In1997afirst analysis of the underlying numerical model has been performed.This subtheme coordinates a number of research ac-tivities in analysis of nonlinear partial differential equations and in mathematical modelling offlow and transport through porous media.The character of the research ranges from very applied to theoretical.An example of an applied activity is the NAM-project, where software was developed to study the mixingof gases in underground reservoirs.An example of a theoretical activity is the collaboration withH.W.Alt(Universit¨a t Bonn),which involves a de-tailed study of a free boundary problem with a cusp. This project participates in the interaction platform ‘Nonlinear Transport Phenomena in Porous Media’, which brings together researchers from TUD,RUL, LUW,RIVM and CWI,and which is supported by the NWO Priority Programme‘Nonlinear Systems’. There are also numerous international contacts.The scientific output in1997includes two Ph.D.theses: Problems in Degenerate Diffusion by Mark Peletier and Multi-Phase Flow Modeling of Soil Contamina-tion and Soil Remediation by Rink van Dijke.PDE RESEARCH–Nonlinear PDEs arising in models for porous mediaflow form the backbone of this project.Particular attention was given to sys-tems consisting of a convection-diffusion equation coupled with an ordinary differential equation.The general case,in which the ODE is in the time vari-able,is treated in the thesis of M.A.Peletier.A par-ticular case,where the ODE is in a space coordinate, appears in a model for salt uptake by mangroves,see Report MAS-R9728.This leads to non-local con-vection,which is shown to imply non-uniqueness.A second activity involves the collaboration Alt-Van Duijn.In a series of papers they study the behaviour of the interface between fresh and salt groundwater in the presence of wells.The interface appears asa free boundary in an elliptic problem.Depending on the pumping rate of the wells,a singularity de-velops in the free boundary in the form of a cusp.A detailed local analysis of the free boundary near such a cusp is presented in Report MAS-R9703.FTPM–This project deals with density drivenflow in porous media.In1997research concentrated on brine transport problems that are related to high-level radioactive waste disposal in salt domes.High salt concentrations give rise to nonlinear transport phenomena such as enhancedflow due to volume (compressibility)effects and the reduction of hydro-dynamical dispersion due to gravity forces.Mainly (semi)analytical techniques(similarity and V on Mi-ses transformations)were used to study the volume effects,see Report MAS-R9724.Report AM-R9616 (Brine transport in porous media:Self-similar solu-tions)has been accepted for publication in Advances in Water ing experimental data of Dr.H.Moser(Technische Universit¨a t Berlin)we also ve-rified a nonlinear dispersion theory proposed by Dr. S.M.Hassanizadeh(Delft University of Technology), which includes the effect of dispersion reduction due to local high salt concentrations.The nonlinear the-ory is in excellent agreement with the experimentalresults,see Report MAS-R9734.We further consi-dered the interface between fresh and salt groundwa-ter in heterogeneous media.This subject is relatedto salt water intrusion problems in coastal aquifers. The interface approximation can be justified when the width of the mixing zone between thefluids is small compared to the vertical extension of the aqui-fer.We studied the resulting set of interface equa-tions numerically,using a moving mesh Finite Ele-ment Method.Moreover,several simplified Dupuit problems were studied and the results were compa-red with FEM solutions,see Report MAS-R9735. NAM–This project deals with the mathematical modelling of gas injection.The dispersion is studied for gas injection into a reservoir.The aim is to un-derstand and quantify the relevant physical processes that lead to mixing of injected gas with residual gas in old reservoirs.The project is sponsored by the NAM(Nederlandse Aardolie Maatschappij).In co-operation with the Faculty of Mining and Petroleum Engineering of the Delft University of Technology a numerical model is being developed at CWI to study the mixing of the gases in detail.NOBIS–Within this project we study soil reme-diation anic contaminants may be removed from the soil either by pumping methods or by injecting air,which enhances biodegradation and volatilization.The correspondingflow of groundwa-ter,organic contaminant and air is described using multi-phaseflow models.Air injection into ground-water(air sparging)in a horizontally layered medium has been studied in Report MAS-R9729.Accurate numerical simulations of the full transient two-phase flow equations were carried out and an almost ex-plicit solution for the steady state airflow just below a less permeable soil layer was derived.The latter solution showed almost perfect agreement with the numerical results when heterogeneity of the layers was increased.To model pumping of a lens of light organic liquid from an aquifer,multi-phase seepage face conditions were applied at the well boundary (Report MAS-R9725).For two different geome-tries of the lens similarity solutions provided good approximations of the removal rate and the location of the remaining contaminant as a function of time. The above results and other work on behaviour ofa lens of organic contaminant and on air sparging have been gathered in Rink van Dijke’s Ph.D.thesis:‘Multi-phaseflow modeling of soil contamination and soil remediation’,which was defended at Wage-ningen Agricultural University on December5,1997. NWO-NLS–This is the Ph.D.project‘Mathemati-cal Analysis of Dynamic Capillary Pressure Relati-ons in Porous Media Flow’.It started in November 1997,with the employment of C.M.Cuesta.It is supported by the NWO Priority Programme‘Nonli-near Systems’.The aim is to study PDEs with higher order mixed derivatives.Such equations arise in mo-dels for unsaturated groundwaterflow,taking into account dynamic capillary pressure.In1997two different subjects have been studied. Report MAS-R9721contains the results of an inves-tigation to the stability of approximate factorization for-methods.Approximate factorization seems for certain multi-space dimensional PDEs a viable alter-native to time-splitting as a splitting error is avoided. The investigation,however,has revealed limitations of the approximate factorization technique with re-gard to numerical stability.The second subject con-cerns RKC(Runge-Kutta-Chebyshev),an explicit time integrator specifically suitable for multi-space dimensional parabolic PDEs.In RKC the stability limitation inherent in explicit methods is greatly re-duced by the use of a three-step Chebyshev recur-sion.The current study has specifically dealt with the development of a production-grade code for non-experencied users.The work has been carried outin co-operation with Prof.L.Shampine,University of Dallas.Details are given in Report MAS-R9715. This report has been accepted for publication in the Journal of Computational and Applied Mathematics. Mini-symposium on Numerical Analysis,Wage-ningen,April3–anizer:P.M.de Zeeuw. Speakers:W.Hundsdorfer(Stability of the Doug-las Splitting Method),E.J.Spee(Advectieschema’s op een Bol voor Atmosferische Ttransport Model-len).Meeting of the Steering Committee of the ESF-Programme‘Free Boundary Problems,Theory and Applications’,CWI,anizer:C.J.van Duijn.TASC Symposium7,CWI,anizers: J.G.Verwer and J.Kok.Speakers:P.J.H.Builtjes (MEP-TNO)(Atmospheric Transport-chemistry Modelling and HPCN),J.G.Blom(LOTOS,a3D Atmospheric Air Pollution Model),J.Kok(Por-ting Atmospheric Transport-Chemistry Software to the NEC SX–4),K.Dekker(TUD)(Modification of Flow Fields to Recover the Property of Divergence Freedom),G.S.Stelling(WL)(NonhydrostaticPressure in Free Surface Flows)and B.P.Som-meijer(Recent Progress in an Implicit Shallow Water Transport Solver).Colloquium‘Flow and Transport in Porous Me-dia’,CWI,September10.Speakers:G.Dagan (Tel-Aviv)and S.E.A.T.M.van der Zee(LUW). Organizers:R.J.Schotting and C.J.van Duijn. TASC Symposium8(‘HPCN-Platformdag’),CWI, anizers:J.G.Verwer andJ.Kok.Speakers:J.G.Verwer(Het TASC Pro-ject HPCN voor Milieutoepassingen),M.van Loon(MEP-TNO)(Langetermijnsimulatie van Ozon),J.G.Blom(Rekenen aan Ozon),B.P.Som-meijer(Simulatie van Transport in Ondiep Water), E.A.H.V ollebregt(TUD)(Parallelle Software voor Stromings-en Transportmodellen)and G.S.Stel-ling(WL)(Simulatie van Afvalwaterlozingen). Mini-symposium on Partial Differential Equati-ons at SciCADE97–International Conference on Scientific Computation and Differential Equations, Grado,September15–anizer:J.G.Ver-wer.Speakers:K.Dekker(TUD)(Parallel GM-RES and Domain Decomposition),W.Hundsdor-fer(Trapezoidal and Midpoint Splittings for Initial Boundary-value Problems),B.P.Sommeijer(RKC, an Explicit Solver for Parabolic PDEs)and J.M. Hyman(Los Alamos)(Minimizing Numerical Er-rors Introduced by Operator Splitting Methods) Colloquium‘Flow and Transport in Porous Me-dia’,CWI,September24.Speakers:A.de Wit (Brussels)and R.J.Schotting(CWI).Organizers: R.J.Schotting and C.J.van Duijn.Workshop‘Interfaces and Parabolic Regularisa-tion’,Lorentz Center(RUL),November5–7.In-ternational workshop with25speakers anizers:J.Hulshof and C.J.van Duijn.MAS Colloquium,CWI,anizer: C.J.van Duijn.Speakers:C.N.Dawson(UT at Austin)(Dynamic Adaptive Methods for Chemi-cally Reactive Transport in Porous Media),P.Wes-seling(TUD)(Numerical Solution of Hyperbolic Systems with Nonconvex Equation of State)and W.A.Mulder(Shell Rijswijk)(Finite Differences and Finite Elements for Seismic Simulation). TASC Symposium9,CWI,ani-zers:J.G.Verwer and J.Kok.Speakers:A.Peter-sen(IMAU)(More Efficient Advection Schemes for the Global Atmospheric Tracer Model),H.Elbern (EURAD)(A Parallel Implementation of a4D-variational Chemistry Data Similation Scheme), E.J.Spee(Rosenbrock Methods for Atmospheric Dispersion Problems)and M.Krol(IMAU)(The TM3Model:Numerical Aspects of Atmospheric Chemistry Aplications).2nd Annual Meeting MMARIE Concerted Action, Barcelona,January15–17:B.P.Sommeijer(Do-main Decomposition for an Implicit Shallow-water Transport Solver).Meeting of the DFG Panel for the Sonderforsbe-reich1578,M¨u nchen,January16–17:Participa-tion by C.J.van Duijn.Meeting of the Scientific Council of the Weier-strass Institut f¨u r Angewandte Analysis und Sto-chastik,Berlin,January24:C.J.Van Duijn partici-pates and is elected vice-chairman of this council. Guest Lectures at the University of Amsterdam, within the framework of the course‘Parallel Scientific Computing and Simulation’,February21 and26:B.P.Sommeijer(Parallel ODE solvers). Harburger Sommerschulen,TU Hamburg-Harburg, February24–28:J.G.Verwer invited speaker (three lectures on the Method of Lines). Universidad Complutense de Madrid,Madrid, March19–23:C.J.van Duijn visits J.I.Diaz.32e Nederlands Mathematisch Congres,Wage-ningen,April3–4:W.Hundsdorfer(Stability of the Douglas Splitting Method),E.J.Spee(Ad-vectieschema’s op een Bol voor Atmosferische Transport-modellen).Istituto per le Applicazioni del Calcolo‘Mauro Pi-cone’,Rome,April7–11:C.J.van Duijn visits M. Bertsch.1st ERCIM Environmental Modelling Group Workshop on Air Pollution Modelling,GMD FIRST,Berlin,April7–8:J.G.Blom(An Evalua-tion of the Cray T3D Programming Paradigms in Atmospheric Chemistry/transport Problems),J.G. Verwer(A Numerical Study for Atmospheric Che-mistry/transport Problems).Both invited. Measurements and Modelling in Environmen-tal Pollution,Madrid,April22–24:M.van Loon (Data Assimilation for Atmospheric Chemistry Models).22nd General Assembly of the European Geo-physical Society,Vienna,April21–25:B.P.Som-meijer(A Fully Implicit3D Transport-chemistry Solver Combined with Domain Decomposition). NWO Symposium Massaal Parallel Rekenen, Veldhoven,May22:J.G.Verwer invited speaker (High Performance Computing and Environmental Pollutions).。
学生石墨烯文献翻译

石墨烯/聚合物纳米复合材料摘要:石墨烯由于其特殊的电导性、机械性能和大的表面积而具有巨大的科研价值,当加入适当时,这些原子薄碳层可以显著提高主要高聚物的物理性能。
我们首先按照从上到下的战略回顾一下从氧化石墨到石墨烯的生产工艺过程,包括每种方法的优点和缺点。
然后按溶解和熔融的战略即分散化学和加热的方法讨论降低氧化石墨在聚合物中的含量。
对于微粒大小的性质、表面性质和在基体中的离散性的技术分析也有介绍。
我们总结石墨烯/聚合物纳米复合材料的导电性、导热性、机械性能和阻气性。
我们结合石墨烯复合材料的加工和可量测性总结这些观点列出最近的挑战和这些新的纳米复合材料的远景。
1介绍基于炭黑、碳纳米管和层状硅酸盐的聚合物纳米复合材料被用于增强聚合物的机械性能、导电性、导热性和阻气性。
石墨烯极其特殊的物理性能和能溶于多种基本聚合物的结合的发现创造了一类新的聚合物纳米复合材料。
石墨烯是由sp2杂化的碳原子按蜂窝状结构排列成的单层、二维片状结构。
它被誉为其他所有不同维数的石墨碳的同素体的基础材料,例如,石墨(三维碳的同素体)由石墨烯的薄碳片正面向上堆积在一起并且分开距离为3.37A组成。
0维同素体,富勒烯(足球烯),可以想象成单层石墨烯的一部分卷曲成的。
一维碳同素体,碳纳米管和碳纳米带可以分别由单层石墨烯旋转和剪切制成。
实际上,然而,这些碳的同素体,除了碳纳米带,都不是由石墨烯合成的。
石墨是一种天然生成的材料,它最早的记载于1555年在英国的Borrowdale,但是它最早的应用可向前追溯4000年。
在1985年发现富勒烯后于1991年第一次合成单壁碳纳米管。
尽管生产石墨烯纳米片的第一个方法报道可以追溯到1970年,但对存在的单层石墨烯在2004年第一次被生产出来,用微机械剥离的方法从石墨中分离出石墨烯。
杨氏模量为1TPa和极限强度为130GPa,单层石墨烯为测量出来的最强的材料。
它的导热系数为5000W/cm3*KJ,与报道的碳纳米束最高值的上限相一致。
外文译文

用固态碳源生长石墨烯摘要:单层石墨烯作为一种可转移材料在2004年第一次被获得并且引起了物理学家、化学家、材料学家强烈地关注。
很多研究都致力于找到获得大面积单层或双层石墨烯的方法。
最近这种方法已经被找到,是通过在铜或镍基底上化学气象沉积(CVD)甲烷或乙炔。
但是CVD方法仅限于未加工的气体原料,而很难应用于更加广泛的潜在的原料。
在这里我们论证一种方法:利用固态碳源—比如聚合物薄膜或小分子,最低只要800℃就能够在金属触媒基底上生长出大面积、高质量、可控制厚度的石墨烯。
原始石墨烯和掺杂石墨烯都是用这种一步工序在同样的设备上生产的。
正文:石墨烯有着非凡的电学和机械性能在很多应用方面都表现出很好的前景。
现在有很多获得石墨烯的方法。
最原始的机械剥离法可以从高取向性的热分解石墨上获得少量高质量的石墨烯。
液体剥落并还原氧化了的石墨烯已经被用于化学转化获取大量石墨烯。
热处理SiC,用无定形碳和CVD方法已被应用在晶片上生长大尺寸石墨烯。
通过引进Ni和Cu作为CVD生长的基底,石墨烯的尺寸、厚度、质量正在接近工业化使用标准。
然而石墨烯本质上是零带隙材料表现出很弱的二极性;基于石墨烯的二极管表现出和低的“开/关”电流比,因此它们被用于电子器件设计时很像金属。
为了改变石墨烯的费米能级以及利用它的电学和光学属性,给石墨烯掺杂得到n型,p型或混合型掺杂石墨烯一直是我们奋斗的目标。
当前,用固态碳源在金属触媒基底上生长单层原始石墨烯已被论证(图1a)第一种被使用的固态碳源是旋涂的聚合物(聚甲基丙烯酸甲酯)(PMMA)薄膜(~100nm),金属触媒基底是铜薄片。
在最低为800℃最高为1000℃(测试上限)的温度,伴随着还原性气流(H2/Ar)的低压条件下生长10分钟,单层一致的石墨烯就在基底上生成了。
因此石墨烯材料被成功的转移的不同的基底上有更多的特性(见Supplementary Materials and Supplem entary Methods)这种源于PMMA的单层石墨烯的拉曼光谱如图1b所示,这个光谱表征了样品1 cm2范围内大于10个位置的情况。
温敏水凝胶的英语

温敏水凝胶的英语The English Composition on Thermo-Sensitive HydrogelsThermo-sensitive hydrogels have gained significant attention in the field of biomedicine due to their unique properties and potential applications. These intelligent materials possess the ability to undergo reversible phase transitions in response to changes in temperature, making them particularly useful in various biomedical applications.Hydrogels are a class of hydrophilic polymeric networks that can absorb and retain large amounts of water or biological fluids within their three-dimensional structure. Thermo-sensitive hydrogels, specifically, exhibit a temperature-dependent phase transition, which means they can undergo a sol-gel transition as the temperature changes. This property is often referred to as the lower critical solution temperature (LCST) or upper critical solution temperature (UCST), depending on the specific polymer system.One of the most well-known thermo-sensitive hydrogels is poly(N-isopropylacrylamide) (PNIPAAm), wh ich has an LCST around 32°C, close to the human body temperature. Below the LCST, PNIPAAmhydrogels are in a swollen, hydrophilic state, allowing for the incorporation and release of various therapeutic agents. However, as the temperature increases above the LCST, the polymer chains undergo a conformational change, leading to the collapse of the hydrogel structure and the expulsion of water. This temperature-induced phase transition makes PNIPAAm-based hydrogels particularly useful for controlled drug delivery applications.The mechanism behind the temperature-responsive behavior of thermo-sensitive hydrogels, such as PNIPAAm, is related to the delicate balance between hydrophobic and hydrophilic interactions within the polymer network. At temperatures below the LCST, the polymer chains are hydrated, and the hydrogen bonding between water molecules and the polymer's amide groups dominates, leading to a swollen, hydrophilic state. As the temperature increases above the LCST, the hydrogen bonding between water and the polymer becomes weaker, and the hydrophobic interactions between the isopropyl groups of the polymer become more prominent. This results in the collapse of the polymer chains, causing the expulsion of water and the formation of a more compact, hydrophobic structure.The unique temperature-responsive behavior of thermo-sensitive hydrogels has led to their widespread application in various biomedical fields. One of the primary applications is in controlleddrug delivery systems. Thermo-sensitive hydrogels can be used as carriers for therapeutic agents, such as small-molecule drugs, proteins, or even cells. These hydrogels can be designed to release the encapsulated drugs in a controlled manner by responding to the temperature changes in the body. For example, a PNIPAAm-based hydrogel loaded with a drug can be administered in a liquid state at room temperature and then undergo a phase transition to a gel state upon reaching body temperature, effectively trapping the drug within the hydrogel matrix. As the temperature increases further, the hydrogel can undergo a volume phase transition, leading to the release of the drug in a controlled manner.Another important application of thermo-sensitive hydrogels is in tissue engineering and regenerative medicine. These hydrogels can be used as scaffolds for cell growth and tissue regeneration. The temperature-responsive nature of the hydrogels allows for easy administration and in situ gelation, which can facilitate the encapsulation of cells or the delivery of growth factors directly to the site of injury or disease. The hydrogel scaffold can then provide a suitable microenvironment for cell proliferation, differentiation, and tissue formation.Thermo-sensitive hydrogels have also found applications in wound healing and burn treatment. The ability of these hydrogels to undergo a sol-gel transition in response to temperature changes canbe exploited to create wound dressings that can be easily applied in a liquid form and then transition to a gel state upon contact with the body. This can help maintain a moist environment, promote wound healing, and prevent infection.Furthermore, thermo-sensitive hydrogels have been investigated for use in various diagnostic and sensing applications. For instance, they can be designed to incorporate responsive elements, such as enzyme-substrate pairs or antibody-antigen interactions, which can trigger a detectable change in the hydrogel's physical properties in response to the presence of specific analytes or biomarkers.The development of thermo-sensitive hydrogels has also led to advancements in the field of injectable biomaterials. These hydrogels can be designed to be injected in a liquid form and then undergo in situ gelation at the target site, allowing for minimally invasive procedures and the delivery of therapeutic agents or cells directly to the site of interest.Despite the numerous promising applications of thermo-sensitive hydrogels, there are still several challenges that need to be addressed. One of the key challenges is the optimization of the LCST or UCST to match the specific requirements of the target application. Researchers are exploring ways to fine-tune the polymer composition and structure to achieve the desired temperature-responsive behavior. Additionally, the long-term biocompatibility and biodegradability of these hydrogels need to be thoroughly investigated to ensure their safe and effective use in biomedical applications.In conclusion, thermo-sensitive hydrogels have emerged as a versatile class of biomaterials with tremendous potential in the field of biomedical engineering. Their temperature-responsive behavior, coupled with their ability to encapsulate and deliver therapeutic agents, make them a promising platform for a wide range of applications, from controlled drug delivery to tissue engineering and regenerative medicine. As research in this field continues to advance, we can expect to see even more innovative and impactful applications of thermo-sensitive hydrogels in the years to come.。
文献翻译

高选择性电致发光纳米传感器测定岩沙海葵毒素摘要:海洋毒素越来越普遍地存在于世界上的许多领域里,在地中海区域呈现出的一种严重的海洋毒素就是海葵毒素,它是一种毒性很强的海洋毒素,并且频繁存在于各种海产品中。
正是因为它的高强度的毒性给人们生活带来了很多害处,找到出一种高灵敏性的检测方法来对海葵毒素进行定性和定量的检测便成了当务之急。
我们设计出了一种超灵敏的电化学发光传感器来检测海葵毒素,利用反海葵毒素抗体的选择性,碳纳米管的良好的传导性能,和基于荧光的传感器的高效性可以很好的对海葵毒素进行检测。
该传感器可根据检测物的浓度不同而出现不同强度的光信号,可以对蚌类海产品体内的海葵毒素进行定量检测,检测限可以达到2.2μg/kg,比一般的检测方法(如液相色谱-质谱联用分析方法)的检测限低了2个以上的数量级。
由于饮食问题广泛严重影地响到人们的健康,食品安全问题越来越受到政府和社会的重视。
设计研发高选择性的设备来对食物以及食物链中的有害物质进行测定成了当务之急。
岩沙海葵毒素在海洋中的扩散越来越严重,这促使我们去研究一种新型的以电致发光为基础的生物传感器来检测这种有毒物质。
众所周知,海葵毒素和它的类似化合物有很强的毒性,使海洋生物受到污染,从而导致人们在进食海产品后发生中毒事件甚至死亡。
尽管温和水域(如地中海)中的被海葵毒素污染的海产品越来越引起重视,并显见于各种报道,但对这种生物分子的定性和定量的检测并没有一个明确的方法,并且在高效性和选择性上还有明显的局限。
面对这些挑战,我们设计了一种新型的混合型系统来对海葵毒素进行检测,这种混合型系统的分析方法结合了三明治型免疫分析与电致发光分析。
抗体特异性识别其抗原的这种特殊能力与电化学发光分析相结合显出了一些优势,如成本低,分析速度快,方法简便。
这种免疫传感器的灵敏度通过界面传导,光学导电波碳电极和功能化的多壁碳纳米管得到了提高。
事实上,碳纳米管以其显著的电子特性并且可以用作特殊的纳米电极,而使得其成为提高传感器性能的最佳候选。
显微高光谱英语

显微高光谱英语Hyperspectral imaging, also known as imaging spectroscopy, is a powerful technique that combines the principles of spectroscopy and digital imaging to provide detailed information about the composition and properties of a material. In hyperspectral imaging, a large number of narrow spectral bands are acquired for each pixel in an image, allowing for the detection and identification of materials based on their unique spectral signatures.By analyzing the spectral data collected by a hyperspectral imaging system, researchers can identify and classify materials based on their chemical composition, physical properties, and other characteristics. This information can be used in a wide range of applications, including remote sensing, agriculture, environmental monitoring, and medical imaging.One of the key advantages of hyperspectral imaging is its ability to provide detailed and accurate information about the materials being imaged. Unlike traditional imaging techniques that capture only three spectral bands (red, green, and blue), hyperspectral imaging can capturehundreds of spectral bands across the visible and infrared spectrum. This allows for the detection of subtle differences between materials that may not be visible to the naked eye.In addition to identifying and classifying materials, hyperspectral imaging can also be used to quantify the amount of a particular material present in a sample. By analyzing the spectral data, researchers can determine the concentration of specific compounds or elements in a material, providing valuable information for a wide range of applications.Overall, hyperspectral imaging is a versatile and powerful technique that has the potential to revolutionize many fields of science and industry. Its ability to provide detailed information about materials and their properties makes it an invaluable tool for researchers and practitioners alike.高光谱成像,也称为成像光谱学,是一种强大的技术,将光谱学原理和数字成像相结合,提供有关材料组成和性质的详细信息。
酵母发酵生产谷胱甘肽的培养基优化_卞芙蓉

酵母发酵生产谷胱甘肽的培养基优化卞芙蓉,劳兴珍,郑 珩,吴梧桐(中国药科大学生命科学与技术学院,江苏南京210009) 摘 要:目的应用Plackett -Burman 设计和球面对称设计实验,对酵母发酵生产谷胱甘肽的培养基进行优化。
方法首先通过Plackett -Burman 设计方法从10个因素中选择出对发酵产量影响较大的因素,即葡萄糖、酵母膏和半胱氨酸含量,然后用球面对称设计对这3个因素各取5个水平进行优化。
结果最佳培养基组成为:葡萄糖23.64g /L ,酵母膏29.07g /L ,半胱氨酸1g /L ,(NH 4)2SO 42g /L ,蛋白胨5g /L ,KH 2PO 41g /L ,MgSO 41g /L ,NaCl 2g /L 。
在优化条件下,发酵液中谷胱甘肽积累量可达162.3mg /L ,比优化前产量提高约56.2%。
结论证明用Plackett -Burman 设计和球面对称设计寻求菌体积累谷胱甘肽的最佳培养基组分是可行的。
关键词:谷胱甘肽;Plackett -Burman 设计;球面对称设计 中图分类号:TQ92 文献标识码:A 文章编号:1005-1678(2009)03-0184-03Study on the optimal media of glutathione production of Saccharomyces cerevisiaeBIAN Fu -rong ,L AO Xing -zheng ,ZHE NG Heng ,WU Wu -tong(School of Life Scie nce and Tec hnology ,China Pharmaceutic al University ,Nanjing 210009,China ) Abstract :Purpose To study the optimal media of glutathione pr oduction of Saccharomyces c erevisiae by Plackett -Burman design and spherical symmetric design .Methods Firstly ,the three factors were selected :glu -cose ,yeast extract and L -cysteine which can apparently influence the glutathione production from ten factors by Plackett -Bur man design ,and then three factors were optimized through spherical symmetric design .Results The optimal media suc h as 23.64g /L gluc ose ,29.07g /L yeast extract ,1g /L L -c ysteine ,2g /L (NH 4)2SO 4,5g /L peptone ,1g /L KH 2PO 4,1g /L MgSO 4,and 2g /L NaCl were applied .Under the optimal media ,the yield of glutathione in the fer mentation broth was 162.3mg /L ,which was 56.2%higher than before .C onclusion It is available to find the optimal media for glutathione pr oduction by Plackett -Burman design and spherical symmet -ric design .Key words :glutathione ;Plackett -Burman design ;spherical symmetric design 收稿日期:2008-12-16作者简介:卞芙蓉(1984-),女,江苏盐城人,在读硕士研究生;郑珩,通信作者,副教授,E -mail :z hengh18@hot mail .co m 。
吸附模型综述
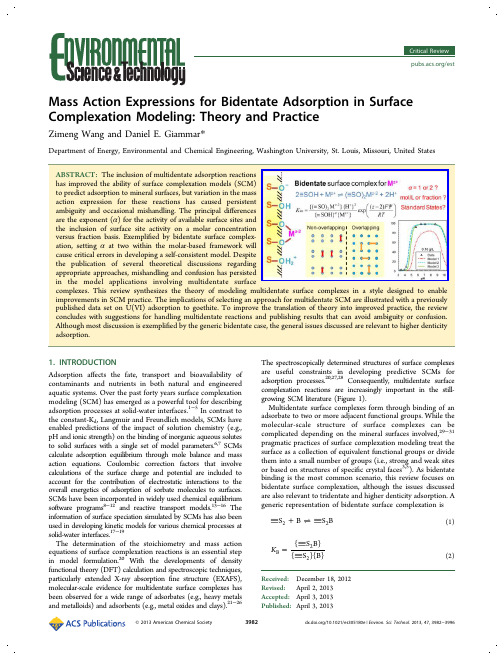
与尘埃等离子体的亲密接触

【创新之路】Way of Innovation专家简介:上世纪80年代之前,尘埃等离子体物理还只是天体物理的小分支,当90年代初期,尘埃等离子体在半导体刻蚀工艺中首次被发现,随后立刻在实验室成功实现后,全球物理学家对该领域表现出高度的热情,引发了一波持续至今的研究热潮,同时一系列重要实验成果不断涌现,苏州大学教授冯岩很荣幸地参与其中。
冯岩向记者解释,尘埃等离子体物理是研究在等离子体环境中纳米到毫米尺度的固体颗粒的一门新兴的交叉学科,研究课题涵盖了等离子体物理、天体物理、半导体加工工艺、凝聚态物理、软物质物理、流体物理、统计物理等。
从2005年5月进入爱荷华大学Goree教授课题组开始接触尘埃等离子体以来,冯岩已有十年尘埃等离子体的研究经历。
冯岩对尘埃等离子体开展的研究,主要以实验为主,同时结合模拟理论研究。
尘埃等离子体的三大新发现尘埃等离子体具备常见的固体和液体的某些性质,像固体的弹性、液体的黏性等。
因此它的黏弹性早有人预言,并已经用于一些理论研究,不过在实验上从未有人对其黏弹性有过真正的量化。
冯岩及其科研团队从系统的剪切流以及应力张量的涨落行为着手,发明了根据颗粒动力学行为而计算出随波数变化的黏度和随频率变化的复数黏度的方法,从而量化其黏弹性,且已成功用于尘埃等离子体实验,系统地从实验上量化了尘埃等离子体在不同空间尺度和时间尺度上的黏弹性。
这一研究很快在尘埃等离子体领域获得广泛好评,冯岩说:“因为这项研究,作为第一作者,我获得了在美国举行的最重要的两个等离子体国际会议,APS-DPP年会和IEEE等离子体会议,特邀报告两次。
”目前,冯岩的这项研究成果已经被别的研究组广泛应用。
尘埃等离子体的多种输运机制也是冯岩的研究内容之一。
在尘埃等离子体实验和模拟中,扩散、黏度和热导这三种输运机制冯岩曾经都系统地研究过,做过一系列课题。
在这其中,他在实验中发现了尘埃等离子体中存在的巨大的黏性致热现象,是目前所有实验体系中最极端的。
石墨烯外国文献翻译

石墨烯基础材料的光电特性Inhwa Jung在这研究报告中,石墨烯基础材料的光电性能被调查,特别是研究具有氧化石墨单层的石墨烯氧化物的物理和化学性质和它的化学简式与石墨的不同。
尽管氧化石墨在一百多年前就被Brodie(在1859年)合成,但直到现在特殊层还没被深入研究,与我们正在研究的石墨烯氧化物比较,物理学家在原始石墨烯(石墨的一个层)发现了卓越的物理输送特性同时也显示石墨烯在纳米电子方面的潜力;这提高我们对包括石墨烯氧化物在内的化学法改变石墨性质的兴趣。
从石墨烯的光学性质方面来看,为了识别和测量石墨烯基底的有效光学性质,由于由硅上的薄介电层组成的基底的作用,一个直截了当的方法被提出。
通过这个方法和优化介电层的厚度,获得石墨烯基底独特晶片和基底的的巨大差别。
选择合适的光学性能和介电层的厚度,氧化石墨的有效折射率和光学吸收系数可以减少氧化石墨,通过对比预测与实际测量的差别可以获得石墨烯。
椭圆光度法成像是一种为光学成像和表征超薄材料(1nm~)例如特殊化学法改变的石墨烯晶片和少层氧化石墨烯晶片保持电势的方法,单独使用椭圆光度法成像无论能否确定它的光学性质和厚度都是非常有趣的,传统的光谱椭圆光度法也可以应用到比特殊晶片宽数毫米的多层叠加的氧化石墨上。
利用两种成像方法得到的结果对比最大的区别在于光学性质的差异。
观察热处理过的单体和多层叠加,多层叠加和单层的区别类似氧化石墨(无论是特殊晶片还是多层叠加)的对比结果。
分别从轮廓仪和AFM得到厚度,解释厚度和光学性质在热处理时会改变的模型被提出。
电学特征是前面提及的异常原始石墨性能基本的技术领域,通过在真空中加热单层石墨氧化物(沉积于基体)对材料的电阻率进行了监测。
通过监测随时间和温度响应的电导率能够表明,导电率的变化可能与一个激活的化学过程有关, 并由此可以获得活化能(势垒高度)。
通过高达85 S/m的时间温度曝光可以知道单层的氧化石墨的导电率,其次在真空中加热并与气相肼发生化学还原可以成倍地得到更高的导电率,如原始石墨一样,氧化石墨导电率对电场方向很敏感,伏安测量还表明,氧化石墨的电气性能与石墨烯存在差别。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
WENO Schemes for Cylindrical and Spherical GridShengtai Li∗Theoretical Division,MS B284,Los Alamos National Laboratory,Los Alamos,NM87545AbstractIn this paper,we apply the high order WENO schemes touniform cylindrical and spherical grid.Many2-D and3-Dproblems can be solved in1-D equations if they have angu-lar and radial symmetry.The reduced equations will typ-ically involve geometric source terms.Therefore,conven-tional numerical schemes for Cartesian grid may not workwell.We propose several approaches to apply the high or-der weighted essentially non-oscillatory(WENO)schemeto the1D cylindrical and spherical grid.We have testedthese schemes with Sedov explosion problem,and havefound that the conservation in multi-dimensional senseis essential to generate physical solutions.The numericalresults show that the globalflux-splitting may fail to workeven for high order WENOfinite-difference schemes.Wehave also shown that only high order WENOfinite-volumeschemes can achieve both the high order accuracy and theconservation.Keyword:PDE,WENO,cylindrical and spherical,Euler equations,Sedov1IntroductionWENO schemes become popular in numerical simula-tions involved discontinuities and sharp fronts.Mostof WENO applications in literatures are for Cartesiangrid.In this paper,we test WENO schemes for othertypes of orthogonal curvilinear uniform grid.We consider a hyperbolic conservation lawu t+∇·f(u)=0,(1)with an initial condition u0.If we know the solutionis radially symmetric,we can rewrite the equationsin polar or spherical coordinates,obtaining a systemthat reduces to a problem in a single space variabler.In this paper,we are particularly interested in theEuler equations.Normally the radially symmetry canbe used to reduce the Euler equations into(ρ)t+1r(rνρu)r=0,∗Email:sli@,Phone:(505)672-4155,Fax:(505)665-5757(ρu)t+1r(rνρu2)r+p r=0,(2)E t+1r(rν(E+p)u)r=0,whereνis a constant that depends only on the di-mension,andν=1for2-D cylindrical grid andν=2 for3-D spherical grid.This system can be rewritten as(ρ)t+(ρu)r=−ν(ρu)/r,(ρu)t+(ρu2+p)r=−ν(ρu2)/r,(3)E t+((E+p)u)r=−ν((E+p)u)/r, which has exactly the same form as the one-dimensional system of equations in Cartesian grid with the addition of a geometric source term on the right-hand side.Even if the real problems of interest must be studied multi-dimensionally,radially symmetric solu-tions are valuable in testing and validating numerical codes.A highly accurate solution to the1-D prob-lem can be computed on afine grid and used to test solutions computed with the multidimensional solver. This is useful not only in checking that the code gives essentially the correct answer in at least some special cases,but also in determining whether the numerical method is isotropic or suffer from grid-orientation ef-fects that lead to the results being better resolved in some directions than in others.Many numerical solvers solve the reduced problem as a1-D problem with a geometric source term(see [6]).This approach has a advantage that the existing numerical solver for the Cartesian grid can be used directly without modification.For the fully discrete methods for conservation law,a Strang splitting[11] strategy is often used to handle the geometric source term separately.For semi-discrete method,where an ODE system is obtained by only space discretization (e.g.,high order WENO schemes),the source term can be incorporated into the ODE system directly. However there is a severe drawback to solve(3) directly.The original multidimensional conservation law is not preserved,i.e.,the numerical schemes may not be conservative in multidimensional sense.Con-servation is a very important requirement in manynumerical schemes to produce physical solution,es-pecially for problems involving shock x and Wendroff[5]has proved that the numerical solution of a conservative and consistent numerical scheme con-verges to the weak solution if it converges as mesh is refined.Many numerical examples have shown that a nonconservative method can fail to converge to a weak solution even if it has high order ter in our numerical tests,we will show that applying the high order WENOfinite-difference schemes directly to the reduced system with the geometric source term yields wrong shock location and strength.To preserve the conservation law,especially to pre-serve the total mass and total energy,we must adapt the numerical schemes to the cylindrical and spheri-cal geometry.For a second order scheme,this adap-tation is easy to implement.However for a higher order(>2nd)scheme,this is not trivial.WENO schemes are based on ENO scheme,which werefirst introduced by Harten,Osher,Engquist,and Chakravarthy[1]in the form of cell averages.The key idea of ENO schemes is to use the smoothest stencil among several candidates to approximate thefluxes at the cell boundaries to a high order accuracy,and at the same time to avoid spurious oscillations near shocks.The cell-averages(finite-volume)version of ENO schemes involves a procedure of reconstruct-ing point values from cell-averages and could become complicated and costly for multi-dimensional ter,Shu and Osher[8]developed aflux ver-sion(finite-difference)of ENO schemes which do not require such a reconstruction procedure.Thefinite-volume schemes and thefinite-difference schemes are equivalent,both in numerical solu-tion and accuracy and in complexity of coding and CPU time,for1-D problem.However,for multi-dimensions,thefinite-volume code becomes much more complicated and costly.Shu[10]pointed that thefinite-volume WENO scheme is about four times more expensive than thefinite difference WENO scheme in2-D,and is about nine times more ex-pensive in3D.However a main restriction on the finite-difference WENO schemes is that the third and higher order of accuracy can only be used on uniform rectangular or smooth curvilinear grid.Even for the smooth curvilinear grid,thefinite-difference WENO schemes have a risk to lose the conservation.We will show it in the next section and in numerical tests. The reconstruction of the point value from cell-average values is the key for both thefinite-difference and thefinite-volume WENO schemes.Thefinite-volume WENO scheme reconstructs the conservative variables at cell-interface,given the cell-average val-ues of them.Thefinite-difference WENO scheme does not have cell-average values explicitly and hence it takes the point value of theflux at each grid point as a cell-average value of someflux function.Shu [10]have given an excellent review and comparison between these two types of schemes.The outline of the paper is as follows.In section2, we propose and compare several approaches to extend the WENOfinite-difference scheme to cylindrical and spherical grids.The high order WENOfinite-volume schemes are constructed in section3.Finally,the numerical schemes are tested and compared by using the Sedov explosion problem.2High Order WENO Finite-Difference SchemesConsider1-D scalar hyperbolic conservation law,u t+f(u)x=0.(4) The WENO schemes for a uniform Cartesian grid can be written asdu jdt+f j+12−f j−12h=0(5)where f j+12is the numericalflux at cell interface,and h is local grid spacing.Wefirst consider thefinite-difference WENO schemes,since it is easily extended to multi-dimensions.2.1Extension to cylindrical andspherical gridIn thefirst approach,we can solve the equations with geometric source term,such as(3).Since the convec-tion term is exactly the same as that in Cartesian grid,the WENO schemes can be plugged in imme-diately.If we denote the geometric source term to be g(r,u),then the WENOfinite-difference scheme yields,du jdt+f j+12−f j−12h=g(r j,u j),(6) where g(r j,u j)=−(f j)/rνj.This scheme has a severe drawback.It does not preserve the original multi-dimensional conservation law,which isR0u(t,r)rνdr= R0u(0,r)rνdr,(7)Applying the midpoint rule to(7)yieldsNj=0u n j1ν+1(rν+1j+12−rν+1j−12)=Nj=0u0j1ν+1(rν+1j+12−rν+1j−12)(8) where u is the volume average value of u at cell[r j−12,r j+12].In a conservative numerical scheme,wecan replace u j with point value u j.It is clear that the WENO discretization(6)is not conservative in the sense of(8).The second approach is to do coordinate transfor-mation x=1ν+1rν+1.Then we obtain∂(f(u)rν)r∂r =∂(f(u)rν)∂x.(9)We can extend the high order WENO scheme to(9) since the grid in x is a smooth curvilinear grid.De-note˜f(x,u)=f(u)rν=f(u)((ν+1)x)νν+1.Then as suggested by Shu[10],the WENO scheme for(9)will have form∂(f(u)rν)rν∂r =˜fj+12−˜f j−12rνj dr,(10)where˜f j+12is the numericalflux at the interface foruniform grid r.Although(10)does not satisfy con-servative form(8),it satisfies an approximate con-servation law, N j=0u j rνj dr=const,which is also important to have the solution converge to the weaksolution.Unfortunately,this approach does not workwell near the origin(r=0).It might be because thattheflux f(u)isflattened by the scaling factor rν,andit has large error near the origin r=0.In numericalexperiments,we found the pressure became negativeafter a short time near the origin.The third approach is to use theflux generatedfrom the Cartesian grid directly and modify it foruse of cylindrical and spherical geometry asdu dt +rνj+12f j+12−rνj−12f j−12dV j=0(11)where dV j(ν)=11+ν(r1+νj+12−r1+νj−12)is the local controlvolume.For the discretization of momentum equa-tion,there is additional source termνp/r on the right side of the equation.To be consistent with the con-servation law in multi-dimensions,this term must be discretized as p j·dV j(ν)/dV j(ν−1).We will show that the numerical scheme(11)is of only second order even if the numericalflux f j+12 calculated as in(5)is of higher order.Taking the spherical geometry as an example,at afixed r=r j, we haver2j+12f j+12−r2j−12f j−12r3j+12/3−r3j−12/3− f j+12−f j−12h+2f j r j =O(h2) Therefore,(11)is only of second order.2.2Flux-splitting forfinite-differenceschemesFor achieving numerical stability and for avoiding en-tropy violation solutions,upwind andflux-splitting approaches are used in constructing the WENOflux. Theflux is written as a sum of the positive and neg-ativefluxes,f±(u),f(u)=f+(u)+f−(u),where f+has positive eigenvalues and f−has nega-tive eigenvalues.There are several choices in defin-ing the splitting.A simple choice is given by the Lax-Friedrichs splitting,which produces very smooth fluxes,f±(u)=12(f(u)±αu),whereαis taken as max u|f (u)|over the relevant of u.If the range is locally defined,it is called the local Lax-Friedrichs(LLF)splitting;if the range is global, it is called the global Lax-Friedrichs splitting(LF). For lower order schemes the quality of the solution is usually very sensitive to the choice of the splitting, and the Lax-Friedrichsflux is very diffusive.But it is claimed in[3]that this sensitivity is much less impor-tant for a higher-order method.It is recommended in [4]that the global LF be used forfifth order WENO scheme.In numerous examples of Cartesian grid,we have not encountered thefifth order WENO scheme failed with the global LF.We have pointed out in[2] that LLF is required to achieve the conservation law strictly in adaptive mesh method and parallel com-putation via domain decomposition.Even in those cases,we found that a global LF can give reasonable ter we willfind that this may no longer be true for spherical and cylindrical grid.We remark that the LF may fail in other cases where the globalαis far more larger than the local αnear the shock and other discontinuities.We have investigated the possibility of develop-ing conservative high orderfinite-difference WENO schemes of form(11).As indicated in Shu[10],the high order WENOfinite-difference schemes can be constructed only for smooth non-uniform mesh.Ourmesh in x=1νrνsatisfies this condition.How-ever,the high order WENO scheme is constructed for∂(f(u)rν)∂r only.The numerical example showed thatthe scheme(10)is no better than other discretiza-tions.3High Order WENO Finite-Volume Scheme3.1Finite-Volume discretizationThe high order WENOfinite-volume scheme of form (11)can be developed following the way of Liu et al.[7].Forfinite-volume scheme,we have cell-average values at each cell,defined byu j(·,t)=1dV r j+12r j−12u(r,t)rνdr.Integrate the hyperbolic systemu t+∂rνf(u)rν∂r=0over each cell,we obtainu t=−1dV(rνj+1f(u(r j+12,t))−rνj−1f(u(r j−12,t))).To achieve the high order accuracy,we need to eval-uate u(r j+1,t)at interface r=r j+1to high order. First,from the given cell average u={u j},we can reconstruct a piecewise high order interpolation poly-nomial R(r).Next the u(r j+12,t)can be evaluated tohigh order by R(x)locally.Theflux at the interface then is evaluated by a numericalflux(approximate or exact Riemann solvers).For example,one could use the simple Lax-Friedrichsflux,which is given byf(u(r j+12),t)=12(f(u+,t)+f(u−,t)−α(u+−u−)),whereαis taken as the upper bound for the eigen-values of the Jacobian at r j+12,and u+and u−arethe values of u(r j+12)from the right and left side ofinterface.3.2Reconstruction procedure Following the reconstruction procedure of Liu et al.[7],given cell averages{u j},we can immediately eval-uate the point values of the solution’s primitive func-tion W(r)at interfaces W(r j+12),where the primitivefunction is defined asW(r)= r r j −12u(ξ,t)ξνdξ.(12)Note that there is a difference between Cartesian andother orthogonal grids to define the primitive func-tion.However,if we set x=1/νrνthenW(x)= x x j −12u(ξ,t)dξ,(13) which is more like the primitive function defined by Liu et al.[7].Differentiating(13)with respect tox yields,u(x,t)=ddxW(x).Discretizing(13)gives W(x j+12)= j i=j u i dV i.Unlike the conservation law in1-D uniform grid,the local control volume dV i is no longer constant.It depends on the location of the cell.To reconstruct the solution,we interpolation W(x) on each stencil S j=(x j−j1+12,...,x j+j2+12)to obtain a polynomial p j(x),i.e.,p j(x l+12)=W(x l+12),l=j−j1,...,j+j2. Obviously the corresponding polynomial p j(x)ap-proximate the solution u(x,t)to high order.The difference between our reconstructions and those in Liu et al.[7]is that the grid in x is non-uniform in cylindrical and spherical grid.The ENO schemes choose the least oscillation one among all possible stencils.The WENO scheme in-stead is a convex combination of all possible sten-cils.Unlike in the uniform mesh,the optimal weights for the WENO scheme now depend on the local grid spacing in x.For x=rν,we haveC0= r i+h i ifν=2(3r2i+14h2)(3r2i+6r i h+134h2)2i i742i94ifν=3 C1= 2r i−h6r i+3h ifν=2,(6r2i−32r i h+72h2)(3r2i+14h2)(6r2i+32r i h+72h2)(9r2i+274h2),ifν=3The actual weight of the stencil in the convex com-bination can be evaluated by smoothness indicator in each stencil.Here we use the measure taken from Jiang and Shu1997[4],which amounts to a measure on the L2-norms of the derivatives.Again,when we evaluate the smoothness measure,the non-uniformity of the grid should be considered.For the third-order WENO scheme,it yields the same measure as for the uniform grid.However for thefifth-order,the mea-sures are different.We remark that for system of equations,the WENO reconstruction should be performed in local characteristicfields.4Numerical ResultsIn this section,we show some numerical results for Sedov problems.We focus ourselves to the results ofWENO scheme.The reference solutions were calcu-lated with second order TVD methods with veryfine grid.4.1Sedov Explosion ProblemThe Sedov explosion problem(Sedov1959)involves the self-similar evolution of a cylindrical or spherical blast wave from a delta-function initial pressure per-turbation in an otherwise homogeneous medium.To initialize the code,we deposit a quantity of dimen-sionless energy =1into a small region of radiusδr at the center of the grid.The dimensionless pressure inside this volume,P0,is given byP 0=3(γ−1) (ν+2)πδrν+1,whereν=1for cylindrical geometry andν=2 for spherical geometry.In running this problem,we chooseδr to be as large as the width of the ghost cells in order to minimize the effects of the reflection boundary conditions.The density is set toρ=1 throughout the grid and the pressure is set to a small value P0=10−5except in the explosion region.The fluid is initially at rest.Although there is an ana-lytical solution for the reduced1-D problem.It is defined implicitly and not readily available.There-fore,we calculated the reference solutions with very fine grid and a conservative second order method. 4.2Results for cylindrical gridThe WENO scheme of(10)failed at t=0.002424due to the negative pressure.At t=0.002,the solution seems OK but has large difference from the reference solutions,especially near the origin.Thefifth order WENOfinite-difference scheme with a global LF splitting failed to produce steep shock front(see Fig.1)for both conservative(11) and nonconservative version(6).We initially thought that it was due to the steep initial conditions for pres-sure.So we calculated the solution with our conser-vative second order method to time t=0.04and then we advance the solution from t=0.04using the WENO with LFflux-splitting.However,we obtained almost the same results.The WENOfinite-difference scheme with LLFflux-splitting gives steep shock front(see2).However, Without the conservation,the shock front has wrong location.It is interesting to see that the results for the modified conservative WENO scheme(11)match very well with the reference solution although it has only second order accuracy.1234560 0.05 0.1 0.15 0.2 0.25 0.3 0.35 0.4 0.45 0.5densityradiusconservative WENO with LFnonconservative WENO with LFreferenceFigure1:WENOfinite-difference with LFflux-splitting at t=0.051234560 0.05 0.1 0.15 0.2 0.25 0.3 0.35 0.4 0.45 0.5densityradiusconservative WENO with LLFnonconservative WENO with LLFreferenceFigure2:WENOfinite-difference with LLFflux-splitting at t=0.054.3Results for spherical gridThe3-D case is very similar to the2-D case,ex-cept that now thefinite-difference discretization and finite-volume discretization of the source term are no longer the same.Since for the conservative form,the source term is added only to the momentum equa-tion,the discretization of the source term will not affect conservation of the mass and energy.How-ever,we observed that the velocity and pressure is totally wrong near the origin if thefinite-difference discretization of the source term is used.If r is large enough,the difference between these two discretiza-tion is O(h2).Near the origin(r=0),the difference between these two is as large as(O(1/h)).We found that the local maximum sound speed near the shock front is about1.5,while it is about310 near the origin.For the global LFflux-splitting,the flux f±(u)deviated the localflux f(u)so much that it cannot reflect the local characteristics any more. That is why the global LF fails for this example.For other examples we have tested so far,the location of0 1234560.050.10.150.20.25 0.30.350.40.450.5d e n s i t yradiusconservative WENO with LF nonconservative WENO with LFreferenceFigure 3:WENO finite-difference with LF flux-splitting att =0.051234560.050.10.150.20.25 0.30.350.40.450.5d e n s i t yradiusconservative WENO with LLF nonconservative WENO with LLFreferenceFigure 4:WENO finite-difference with LLF flux-splitting att =0.05the global maximum sound speed is always close to the shock front.However,for this example,the den-sity is extremely low (about O (10−5)for 3-D case)near the origin and pressure is relatively large (0.188),the local sound speed calculated by c 2=γp/ρnear the origin is much larger than that near the shock front.We also test the conservative WENO finite-difference scheme (10).If we start from t =0,the integration stops after a few time steps due to the negative pressure.We started the simulation from t =0.01(scheme Eq.(11)is used before t =0.01),the results are in good agreement with the reference solutions except at the origin.Fig.7shows that the high order scheme (10)has almost the same results as the second order scheme (11).Fig.8shows that the scheme (10)has large error near the origin.The pres-sure should be constant near the origin.However,the result from scheme (10)has an incorrect large jump near the origin.-55 10 1520 2530 35 40 45 0 0.0050.01 0.015 0.02v e l o c i t yradiusfinite-volume discretization of source term finite-difference discretization of source termFigure 5:Results of the different discretizations for the source term near the origin.1234560 0.05 0.1 0.15 0.20.25 0.3 0.35 0.4 0.45 0.5d e n s i t yradius3rd order WENO finite-volume schemereferenceFigure 6:Results of the third-order WENO scheme for Sedovproblem.5ConclusionWe tested WENO scheme for radially symmetric cylindrical and spherical grid.Numerical results show that preserving conservation law in the numerical schemes is crucial to have accurate shock location and strength.Traditional implementation to solve the cylindrical and spherical problem with the geo-metric source terms does not preserve the mass and energy,although it can achieve higher than second order accuracy.Treating the cylindrical or spherical grid as smooth nonuniform grid,as WENO finite-difference scheme applied to general curvilinear grid,has large error near the origin so that it failed for some initial conditions.There is also a risk with this approach that the discontinuity will be spread out be-cause the weight in WENO scheme will be smoothed out by the geometric factor (r for 2-D and r 2for 3-D).The modified conservative WENO finite-difference scheme produces good results.However,it is only of second order accuracy in theory.This leads to a question that the complexity and effort of the WENO1234560.26 0.280.3 0.32 0.34d e n s i t yradiusWENO scheme (12)WENO scheme (13)Reference solutionFigure 7:WENO finite-difference with LLF flux-splitting att =0.05.1.81.922.12.22.3 2.42.52.60 0.002 0.0040.006 0.008 0.01p r e s s u r eradiusWENO scheme (12)WENO scheme (13)Reference solutionFigure 8:WENO finite-difference with LLF flux-splitting att =0.05near the origin.may not be worthy for such low order of accuracy.Ac-tually,a second-order TVD scheme may work much efficient in this case.We should mention that the global flux-splitting may not work even for high order method.The spu-rious solution is generated if the global maximum characteristics speed is far more larger than the local speed near the shock or contact.For robustness,we suggest the local flux-splitting method be used.The WENO finite-volume scheme can achieve both the conservation law and the high order accuracy.However,it might be too much costly for multi-dimensional problem.Since we have an orthogonal uniform grid,a low order method with fine grid may work more efficient than the high order WENO finite-volume scheme.References[1] A.Harten,S.Osher, B.Engquist,and S.Chakravarthy,Appl.Numer.Math.,2(1986),347-377.[2]Shengtai Li and Mac Hyman,Adaptive Mesh Refine-ment for Finite Difference WENO Schemes,Tech.Rep.Los Alamos Report LA-UR-03-8927,LANL,2003,[3]G.S.Jiang and C.-C.Wu,put.Phys.,150(1999)561-594.[4]G.S.Jiang and C.W.Shu,p.Phys.126(1996),202.[5]P. x and B.Wendroff,Comm.Pure Appl.Math.,13(1960),217-237.[6]R.Leveque,Finite Volume Methods for HyperbolicProblems ,Cambridge texts in applied mathematics,2002.[7]X.-D.Liu,S.Osher and T.Chan,put.Phys.,115(1994),200-212[8] C.W.Shu and S.J.Osher,put.Phys.,83(1989),32-78.[9] C.W.Shu,put.,5(1990),127-149.[10] C.W.Shu,High order finite difference and finitevolume WENO schemes and discontinuous Galerkin method for CFD,ICASE Rep.2001-11,NASA,2001.[11]G.Strang,SIAM J.Numer.Ana.,5(1968),506-517.。
