加拿大安大略省十年级数学试卷英文
初中全英文数学试卷

Section 1: Multiple Choice (30 points)1. Which of the following is a prime number?A. 25B. 29C. 40D. 332. Solve for x: 3x + 5 = 19A. x = 3B. x = 4C. x = 5D. x = 63. What is the area of a rectangle with a length of 8 units and a width of 5 units?A. 15 square unitsB. 40 square unitsC. 45 square unitsD. 80 square units4. If a car travels at a speed of 60 kilometers per hour for 3 hours, how far will it travel?A. 180 kilometersB. 300 kilometersC. 360 kilometersD. 420 kilometers5. Simplify the expression: 8 - 3(2 + 4)B. 5C. 10D. 146. Solve for y: 2y - 7 = 11A. y = 3B. y = 4C. y = 5D. y = 67. What is the value of x in the equation 4x - 2 = 14?A. x = 3B. x = 4C. x = 5D. x = 68. If a triangle has two sides measuring 6 cm and 8 cm, what is the range of possible lengths for the third side?A. 2 cm to 14 cmB. 4 cm to 12 cmC. 6 cm to 10 cmD. 8 cm to 14 cm9. Simplify the expression: 3(4 - 2) ÷ 2A. 3B. 4C. 510. What is the perimeter of a square with a side length of 7 units?A. 14 unitsB. 21 unitsC. 28 unitsD. 49 unitsSection 2: Fill in the Blanks (20 points)11. The product of two consecutive integers is 42. Find the integers.12. The sum of three numbers is 27. If two of the numbers are 8 and 11, find the third number.13. Solve for z: 5z + 10 = 2514. The area of a circle is 144π square units. Find the radius of the circle.15. If a train travels at a speed of 100 kilometers per hour for 5 hours, what is the distance it will cover?Section 3: Short Answer (25 points)16. Explain how to find the perimeter of a rectangle when given the length and width.17. Solve the following system of equations:2x + 3y = 84x - y = 1218. Convert 0.25 into a percentage.19. What is the meaning of the term "factor" in mathematics?20. Solve the following equation for x: 4x - 3(2x + 1) = 11Section 4: Extended Answer (25 points)21. A bookstore sells books at a discount of 20% off the original price. If the original price of a book is $30, how much will the bookstore charge for the book after the discount?22. A farmer has 200 meters of fencing to enclose a rectangular garden. If the length of the garden is twice its width, what are the dimensions of the garden?23. A school is planning a trip for 30 students. The cost of the trip is $600 for transportation and $10 per student for admission. How much will the school spend in total for the trip?24. Solve the following quadratic equation by factoring: x^2 - 5x + 6 = 025. A triangle has angles measuring 30°, 60°, and 90°. If the shortest side of the triangle is 6 units long, find the lengths of the other two sides.Total Points: 100Good luck!。
英文版初中数学试卷附答案

Section 1: Multiple Choice (30 points, 1 point each)1. Which of the following is a prime number?A) 25B) 29C) 36D) 402. Solve for x: 3x + 5 = 19A) x = 4B) x = 5C) x = 6D) x = 73. What is the value of 8^2?A) 16B) 32C) 64D) 1284. If a triangle has sides of lengths 3, 4, and 5 units, what type of triangle is it?A) EquilateralB) IsoscelesC) ScaleneD) Right-angled5. Simplify: 12 ÷ (4 + 2)A) 2C) 4D) 66. The perimeter of a rectangle is 30 cm. If the length is 10 cm, what is the width?A) 5 cmB) 6 cmC) 7 cmD) 8 cm7. What is the area of a square with side length 6 cm?A) 36 cm²B) 48 cm²C) 60 cm²D) 72 cm²8. Solve for y: 2y - 3 = 7A) y = 2B) y = 3C) y = 4D) y = 59. The ratio of boys to girls in a class is 3:2. If there are 24 girls, how many boys are in the class?A) 12B) 18C) 2410. Simplify: (7 - 3) ÷ 2A) 2B) 1.5C) 1D) 0.5Section 2: Short Answer (40 points, 5 points each)11. Write the prime factorization of 56.12. Solve the equation: 5x - 2 = 3x + 9.13. Find the sum of the first 10 even numbers.14. What is the value of x in the equation: 2(x + 3) = 16?15. Simplify: (9 - 4) × (6 + 2).Section 3: Problem Solving (30 points, 10 points each)16. A train travels at a speed of 60 km/h. How long will it take to travel 240 km?17. A garden is in the shape of a rectangle with a length of 30 m and a width of 20 m. What is the perimeter of the garden?18. A book has 240 pages. If a student reads 40 pages each day, how many days will it take for the student to finish reading the book?Section 4: Extension (10 points)19. Solve the following system of equations:2x + 3y = 8x - y = 2Answers:1. B2. B3. C4. D5. B6. A7. A8. C9. B10. BSection 2:11. 56 = 2^3 × 712. 5x - 2 = 3x + 9 → 2x = 11 → x = 5.513. Sum of first 10 even numbers = 2 + 4 + 6 + ... + 20 = 11014. 2(x + 3) = 16 → x + 3 = 8 → x = 515. (9 - 4) × (6 + 2) = 5 × 8 = 40Section 3:16. Time = Distance / Speed = 240 km / 60 km/h = 4 hours17. Perimeter = 2 × (Length + Width) = 2 × (30 m + 20 m) = 100 m18. Days = Total pages / Pages read per day = 240 / 40 = 6 days Section 4:19.2x + 3y = 8x - y = 2From the second equation, x = y + 2.Substitute x in the first equation:2(y + 2) + 3y = 82y + 4 + 3y = 85y = 4y = 0.8Substitute y back into the second equation: x = 0.8 + 2x = 2.8Solution: x = 2.8, y = 0.8。
国际学校十年级数学期末测试卷(英语)

2. If cos q = 0.6691306, then q = a) 48° b) 42° c) 33.8° d) 22.9°
3. If DABC ~ DPQR, then a) AB = PQ b) A = P c) a = b d) c = r
Final Practice Exam
4. The cost of 4 L of milk is $7.35. The unit cost per litre is a) $3.68 b) $1.84 c) $2.45 d) $0.735
5. A 10% discount off an item priced at $30.00 would save you a) $3.00 b) $27.00 c) $10.00 d) $1.00
8. A rotation of a shape is a) a slide b) a translation c) a turn d) a mirror image
9. Which statement is true about angles with parallel lines with a transversal? a) All angles are 60° b) Interior alternate angles are complementary c) Corresponding angles are supplementary d) Exterior alternate angles are congruent
Final Practice Exam
3
4
Grade 10 Essential Mathematics
加拿大数学十年级(高一)省考模拟试题A

PART A: MULTIPLE-CHOICE QUESTIONS (calculator not permitted)
Value: 12 marks
Suggested Time: 30 minutes Allowable Time: 40 minutes
Page 1
Use the following graph to answer question 2.
y
10
5
–10
–5
–5
x
5
10
–10
2. Which of the following equations describes the linear relation graphed above?
Do not access your calculator until directed by the supervisor. At the end of the 40 minutes, the supervisor will give you permission to access your calculator.
where n is the number of students attending the prom. Calculate the cost of renting the hall if 70 students attend. A. $108 B. $500 C. $780 D. $970
6. Which of the following statements are true?
Contents: 31 pages 54 multiple-choice questions (maximum of 54 marks) 6 numeric-response questions (maximum of 6 marks)
加拿大安大略地区十学年数学试卷英文

GRADE 10 PRINCIPLES OF MATHEMATICS (ACADEMIC)MPM 2DTotal Marks:INSTRUCTIONS:1. Calculators may be used.2. Read all instructions carefully in order to maximize your mark.A/C[K] Part A – Multiple Choice 25 Marks (25 questions * 1 mark each)For each of the following questions in this section, circle the letterrepresenting the correct answer.1. A linear system of two equations that has one solution represents twolines that are:a) parallel b) coincident c) intersecting d) none of these2. The midpoint of RS is M(8, -1). If point S has coordinates (11, 4) what are the coordinates of point R ? a) (3, -6)b) (15, -6)c) (5, -6)d) (3, 9)3. The midpoint of the line segment with end points A(-8, 8) and B(6, 4) is: a) (0, 10)b) (1, 2)c) (7, 2)d) (-1, 6)4. The equation of a horizontal line passing through the point (4, 2) is: a) 2=xb) 4=yc) 2=yd) 4=x5. The equation of a line with a slope of 5=m and a y intercept of 8 is: a) 85+=x yb) 85+-=x yc) 85--=x yd) 58+=x y6. The slopes of 2 lines are -7 and 71. These lines are said to be:a) parallelb) perpendicularc) coincidentd) none of these7. The slope of a line segment passing through 2 points (10,- 4) and (-2, -16) is: a) 1b) 2c) -1d) -28. The length of a line segment with end points (-6, 7) and (-1, -5) is: a) 12b) 5c) 13d) 1699. The diameter of a circle whose equation is 28922=+y x is:a) 15b) 16c)17d) none of these10. T he equation of a circle with a centre of (0, 0) that also passes through the point (-8, -6) is: a) 1022=+y xb) 10022=+y xc) 1422=+y xd) 4822=-y x11. T he y-intercept of the line 01052=+-y x is: a) 2b) -2c) 10d) 512. T he slope of the line 0124=-+y x is: a) 2b) -2c) 1d) 013. I f (-3, y) is a solution to the equation 132=+y x , what is the value of y ? a) 3b) 6c) 5d) 814. T he product ()()z y x z y x 323243-- is equal to:a) 2612z xyb) 26412z y xc) 2612z xy -d) 00412z y x15. A simplified expression for ()()n m n m ----52 is: a) m 7b) n m 27+c) m 3-d) n m 27-16. A simplified expression for 242927abcbc a -- is:a) ac 3 b) abc 3 c) 23acd) 223c a17. T he slope of the line, which is perpendicula r to the line, 084=+-y x is: a) -4 b) 4 c) 1 d) -118. T he shortest distance from the point (2, -3) to the line 4-=x is: a) 5 b) 3 c) 2 d) 619. T he value of the polynomial 8542+-a a when 3-=a is: a) 59 b) 44 c) 13 d) 2920. W hich of the following is not a function : a)()()(){}7,6,5,4,3,2b) 22x y =c) 22y x =d) ()()(){}3,8,3,7,2,621. T he range of the relation whose equation is 52--=x y is: a) 5-≤y b) 5≤y c) 5-≥y d) 5≥y22. T he vertex of the parabola ()642--=x y is:a) ()6,4- b) ()6,4- c) ()4,6- d) ()4,6-23. T he equation of the axis of symmetry of the parabola ()5242+--=x y is:a) 5=x b) 5-=x c) 2=xd) 2-=x 24. A parabola with a vertex of ()3,2 and a stretch factor of 41- (relative to2x y =) would have an equation of: a) ()32412+--=x y b) ()32412++-=x y c)()23412-+-=x y d) ()23412++-=x y25 The parabola k x y +-=24 passes through the point ()3,2-. T he value of k is: a) -19b) 11c) 13d) 19A/CPart B – Short AnswersFor each of the questions in this section, write your answers in the spaces provided . Use the foolscap provided for any rough work. Show details of calculations wherever requested.1. In the accompanying diagram, state each of the following: (4 Marks) [K] a) domain: __________ (1 Mark)[K]b)range: __________ (1 Mark)[C]c)Is the relation a function? Justify your answer. (2 marks)[A] 2. The x-intercepts of the parabola 2892-y are: __________ and=x__________.(Show your work) (2 Marks)[A] 3. The roots of the quadratic equation 032=1710x are: __________ and+-x__________.(Show your work) (3 Marks)[A] 4. Write the equation of the parabola with a vertex of (4, 23) if it passesthrough the point (-1, -2): (Show your work) (3 Marks)____________________[T] 5. A line passes through 2 points (1, 4) and (2,-4). Calculate the slope of the line. Also show the equation of the line in the form 0ByAx. (Show+=+Cyour work) (4 Marks)____________________ ____________________Slope Equation[K] 6. The Tangent of 45 is: __________ (1 Mark)[A] 7. a) In the accompanying diagram, the two triangles are similar. What is thevalue of x?(Show your work) (2 Marks) Array=x__________[T] b) If the area of the smaller triangle is 8 cm 2, what is the area of the larger triangle?(Show your work) ( 2 Marks)Area = __________[K]8 Given that sin A = 21, find A ∠ (to the nearest degree) __________ (1Mark)[A] 9. In the accompanying right triangle , find the value of x to one decimal place.(Show your work) (2 Marks)=x ________[A] 10. U se the SINE LAW to find the value of side x to one decimal place.(Show your work) (2 Marks)3028︒x56︒42︒x30x = ________[A] 11. U se the COSINE LAW to find the value of side x to one decimal place.(Show your work) (2 Marks)x = ________[T] 12. F actor each of the following to the fullest extent possible: (4 Questions * 2 marks each)a) y x my mx 22--+________________________b) 31142--x x________________________c) 2416916y x -________________________56︒2030xd) 2225rs-________________________ r+9s30A/C Part C – Full Solutions RequiredFor each of the questions in this section, full solutions are required.Record your answers in the spaces provided. Use the foolscap providedfor any rough work.[A] 1. Solve the linear system using the elimination method. Remember tofind values for both x and y. (5 Marks)22+yx5=-yx=32-21[C] Explain what the solution above represents geometrically. How do youknow that the solution you arrived at is the correct answer? (2 Marks)[A]2. Expand and simplify the polynomial ()()()21432+-+-x x x . (4 Marks)[T]3. Find the equation of the line perpendicular to the line 088=-+y x and passing through the point (-4, 1). (4 Marks)[T] 4. From the window of one building, a man finds that the angle ofelevation to the top of a second building is 47︒ and the angle ofdepression to the bottom of the same building is 58︒. The buildings are 60 m apart. Find the height of the 2nd building to the nearest metre.A diagram is required. (6 Marks)[T] 5. ABC has vertices A(1, 7), B(-5, 3) and C(3, -1). Determine the equation for AE, the altitude from vertex A to the opposite side BC.(5 Marks)6. The hypotenuse of a right triangle is 26 cm. The sum of the other twosides is 34 cm. (9 Marks)[T] a) Find the length of the other two sides of the triangle. (3 Marks)[T] b) Find the measure of the other two angles. Round to the nearest degree. (3 Marks)[C] c) Describe a situation where you would be able to use knowledge ofthe Pythagorean theorem in a practical, real life situation. (3 Marks)[T] 7. A rectangular skating rink measures 20m by 20m. It has been decided to increase the area of the rink by a factor of 4. Determine how mucheach side should be extended. Assume that each side is extendedby the same amount. (6 Marks)[C]What is the significance of keeping the skating rink in the shape of a square? Justify your answer. (3 Marks)[A]8. a) Solve 35122+=d d using the quadratic formula. (2 Marks)[A] b) Solve 03122=-x by factoring. Check your solutions. (2 Marks)。
加拿大数学十年级(高一)省考模拟试题B汇编

You have Examination Booklet Form B. In the box above #1 on your Answer Sheet, fill in the bubble as follows.
Exam Booklet Form/ A B C D E F G H Cahier d’examen
A. decreased by 15 km h B. decreased by 5 km h C. increased by 15 km h D. increased by 11 km h
3. Solve the following system of equations:
4x + 2y = 8 3x + y = 1
13. Marbles are placed in a jar one at a time. Which graph below best represents the total mass of the jar and marbles as the marbles are added?
A.
B.
Total Mass
INSTRUCTIONS: No calculator may be used for this part of the examination. For each question, select the best answer and record your choice on the blue Answer Sheet provided.
Foundations of Mathematics and Pre-Calculus 10 – 2010–2011 Sample B
Page 5
PART B: MULTIPLE-CHOICE QUESTIONS (calculator permitted)
加拿大安大略省十年级数学试卷 英文

INSTRUCTIONS:1.Calculatorsmaybeused.2.Readallinstructionscarefullyinordertomaximizeyourmark.A/C [K]PartA –MultipleChoice25Marks(25questions*1markeach)Foreachofthefollowingquestionsinthissection,circletheletter representingthecorrectanswer. 1. A linear systemoftwoequationsthathasonesolutionrepresentstwolinesthatare: a) parallel b) coincident c) intersecting d) noneofthese 2. ThemidpointofRSisM(8,-1).IfpointShascoordinates(11,4)whatarethe coordinatesofpointR ? a) (3,-6) b) (15,-6) c) (5,-6) d) (3,9) 3. The midpoint ofthelinesegmentwith endpoints A(-8,8)andB(6,4)is: a) (0,10) b) (1,2) c) (7,2) d) (-1,6) 4. The equation ofahorizontal line passingthroughthepoint(4,2)is: a) 2=x b) 4=y c) 2=y d) 4=x 5. The equation ofalinewitha slope of 5=m anda yintercept of8is: a) 85+=x y b) 85+-=x y c) 85--=x y d) 58+=x y6. The slopesof2linesare-7and 71.Theselinesaresaidtobe:a) parallel b) perpendicular c) coincident d) noneofthese 7. The slope ofalinesegmentpassingthrough 2points (10,-4)and(-2,-16)is: a) 1 b) 2 c) -1 d) -2 8. The length ofalinesegmentwith endpoints (-6,7)and(-1,-5)is: a) 12 b) 5 c) 13 d) 169 9. The diameter ofacirclewhose equation is 28922=+y x is: a) 15 b) 16 c)17 d) noneofthese 10. The equation ofacirclewitha centreof(0,0)thatalsopassesthroughthe point(-8,-6)is: a) 1022=+y x b) 10022=+y x c) 1422=+y x d) 4822=-y x 11. The y-intercept oftheline 01052=+-y x is: a) 2 b) -2 c) 10 d) 5 12. The slope oftheline 0124=-+y x is: a) 2 b) -2 c) 1 d) 0 13. If (-3,y)isasolution totheequation 132=+y x ,whatisthevalueofy ? a) 3 b) 6 c) 5 d) 8 14. The product ()()z y x z y x 323243--isequalto: a) 2612z xy b) 26412z y x c) 2612z xy - d) 00412z y x 15. A simplifiedexpression for ()()n m n m ----52is: a) m 7 b) n m 27+ c) m 3- d) n m 27-16. A simplifiedexpression for 242927abc bc a --is:a) ac 3 b) abc 3 c) 23ac d) 223c a 17. The slope oftheline,whichis perpendicula rtotheline,084=+-y x is: a) -4 b) 4 c) 1 d) -118. The shortestdistance fromthepoint(2,-3)totheline 4-=x is: a) 5 b) 3 c) 2 d) 6 19. The value ofthepolynomial 8542+-a a when 3-=a is: a) 59 b) 44 c) 13 d) 29 20. Whichofthefollowingis notafunction : a) ()()(){}7,6,5,4,3,2 b) 22x y = c) 22y x = d)()()(){}3,8,3,7,2,621. The range oftherelationwhoseequationis 52--=x y is: a) 5-≤y b) 5≤y c) 5-≥y d) 5≥y 22. The vertex oftheparabola ()642--=x y is: a) ()6,4- b) ()6,4-c) ()4,6-d) ()4,6-23. The equation ofthe axisofsymmetry oftheparabola ()5242+--=x y is: a) 5=x b) 5-=x c) 2=x d) 2-=x24. Aparabolawitha vertexof ()3,2anda stretchfactorof 41-(relativeto 2x y =)wouldhaveanequationof:a) ()32412+--=x y b) ()32412++-=x y c)()23412-+-=x y d) ()23412++-=x y25 The parabola k x y +-=24passesthroughthepoint ()3,2-.T hevalueof k is: a) -19 b) 11 c) 13 d) 19A/C PartB –ShortAnswersForeachofthequestionsinthissection,writeyouranswersinthespacesprovided .Usethefoolscapprovi dedforanyroughwork.Showdetailsofcalculationswhereverrequested.1. Intheaccompanyingdiagram,stateeachofthefollowing:(4Marks) [K] a) domain:__________(1Mark) [K] b) range:__________(1Mark)[C]c)Istherelationafunction?Justifyyourans wer.(2marks)[A] 2. The x-intercepts oftheparabola 2892-=x y are:__________and__________. (Showyourwork)(2Marks)[A] 3. The roots ofthequadraticequation 0101732=+-x x are:__________and__________. (Showyourwork)(3Marks)[A] 4. Writethe equationoftheparabola withavertexof(4,23)ifitpassesthroughthepoint(-1,-2):(Showyourwork)(3Marks) ____________________ [T]5. Alinepassesthrough2points(1,4)and(2,-4).Calculatetheslopeoftheline .Alsoshowtheequationofthelineintheform 0=++C By Ax .(Showyour work)(4Marks) ____________________ ____________________ Slope Equation [K]6. TheTangentof 45is:__________(1Mark)[A] 7. a)Intheaccompanyingdiagram,thetwotrianglesaresimilar .Whatisthevalueof x ? (Showyourwork)(2Marks) =x __________ [T]Area=__________[K] 8 GiventhatsinA=21,find A ∠(tothenearestdegree)__________(1Mark)[A] 9. Intheaccompanying righttriangle ,findthevalueofxtoonedecimalplace.(Showyourwork)(2Marks)=x ________[A] 10. Usethe SINELAW tofindthevalueofsidextoonedecimalplace. (Showyourwork)(2Marks)x=________[A] 11. Usethe COSINELAW tofindthevalueofsidextoonedecimalplace. (Showyourwork)(2Marks)x=________[T] 12. Factor eachofthefollowingtothefullestextentpossible:(4Questions*2markseach) a) y x my mx 22--+ ________________________ b) 31142--x x ________________________ c) 2416916y x - ________________________d) 2225309s rs r +- ________________________A/C PartC –FullSolutionsRequiredForeachofthequestionsinthissection,fullsolutionsarerequired.Recordyouranswersinthespacesprov ethefoolscapprovidedforanyroughwork. [A] 1.Solvethelinearsystemusingthe eliminationmethod .Remembertofindvaluesforbothxandy.(5Marks) [C]Explainwhatthesolutionaboverepresentsgeometrically.Howdoyouknowthatthesolutionyouar rivedatisthecorrectanswer?(2Marks)[A] 2. Expandandsimplify thepolynomial ()()()21432+-+-x x x .(4Marks)[T] 3. Findthe equation ofthelineperpendiculartotheline 088=-+y x andpassingthroughthepoint(-4,1).(4Marks) [T]4.Fromthewindowofonebuilding,amanfindsthattheangleofelevationtothetopofasecondbuildin gis47?andtheangleofdepressiontothebottomofthesamebuildingis58?.Thebuildingsare60mapart.Findtheheightofthe2nd buildingtothenearestmetre.Adiagramisrequired.(6Marks) [T] 5. ?ABChasverticesA(1,7),B(-5,3)andC(3,-1).DeterminetheequationforAE,thealtitudefromvertexAtotheoppositesideBC.(5Marks) 6. Thehypotenuseofa righttriangle is26cm.Thesumoftheothertwosidesis34cm.(9Marks) [T] a) Findthelengthoftheothertwosidesofthetriangle.(3Marks)[T] b) Findthemeasureoftheothertwoangles.Roundtothenearestdegree.(3Marks)[C] c)DescribeasituationwhereyouwouldbeabletouseknowledgeofthePythagoreantheoremi napractical,reallifesituation.(3Marks)[T]7.Arectangularskatingrinkmeasures20mby20m.Ithasbeendecidedtoincreasetheareaoftherinkb yafactorof4.Determinehowmucheachsideshouldbeextended.Assumethateachsideisextende dbythesameamount.(6Marks)30 28?x56? 42?x 3056? 2030 x[C]Whatisthesignificanceofkeepingtheskatingrinkintheshapeofasquare?Justifyyouranswe r.(3Marks)[A] 8. a) Solve3d usingthe quadraticformula.(2Marks)=d5122+[A] b)Solve0x byfactoring.Checkyoursolutions.(2Marks)-3122=。
加拿大数学十年级(高一)省考模拟试题A

PART A: MULTIPLE-CHOICE QUESTIONS (calculator not permitted)
Value: 12 marks
Suggested Time: 30 minutes Allowable Time: 40 minutes
INSTRUCTIONS: No calculator may be used for this part of the examination. For each question, select the best answer and record your choice on the blue Answer Sheet provided.
This is the end of Part A (calculator not permitted).
If there is some time left, you have two options: i) Make sure you have answered all the questions. You will not be able to go back to this section at the end of 40 minutes. ii) You may proceed to the rest of the examination without the use of a calculator; there are many questions that do not require a calculator. Make sure you flag any questions you skip to remember to go back to them later.
加拿大数学10年级练习第四部分几何电子教案

加拿大数学10年级练习第四部分几何1. Find the ratio of the perimeter of the larger rectangle to the perimeter of the smaller rectangle.••••2. Find the area of the triangle with ∠A = 41, b = 5 ft, and c = 4 ft. Round your answer to two decimalplaces.• 6.56 ft2•10.00 ft2•7.55 ft2•13.12 ft23. Find the area of kite ABCD if BD = 48 cm, AB = 25 cm, and BC = 26. The kite is not drawn to scale.•289 cm2•70 cm2•816 cm2•408 cm24. The diameter of a basketball rim is 18 inches. A standard basketball has a circumference of 30 inches.About how much room is there between the ball and the rim in a shot in which the ball goes in exactly in the center of the rim?• 4.2 in.• 4.78 in.•none of these5. Find the area.•718 square units•545 square units•534.5 square units•701 square units6. Name the major arc and find its measure.•m = 275•m = 170•m = 85•m = 2757. Find the area of the regular polygon. Round your answer to the nearest tenth.•40.0 in.2•67.6 in.2•110.2 in.28. Name the minor arc and find its measure.•m = 275•m = 85•m = 170•m = 2759. Find the area of ΔABC. The figure is not drawn to scale.•26.06 cm2•28.00 cm2•22.73 cm2•24.95 cm210. Find the circumference of the circle. Use as an approximation of π.•11 cm•5 cm•6 cm11. If a dart hits the target at random, what it the probability that it will land in the unshaded region?••••12. Find the area of the circle. Use π = 3.14 and round to the nearest hundredth.•91.56 m2•16.96 m2• 5.72 m2•22.89 m213. Find the area of a regular pentagon with side 6 cm.•76.6 cm2•45.2 cm2•123.9 cm214. Find the probability that an object falling randomly on the figure will land in the shaded area.•0.32•0.36•0.5•0.2615. Find the area.•102.9 yd2•205.8 yd2•35.35 yd2•105.5 yd216. Two concentric circles have radii of 14 cm and 24 cm. Find the probability to the nearest thousandth thata point chosen at random from the circles is located outside the smaller circle and inside the larger one.•0.066•0.583•0.01717. A slide that is inches by inches is projected onto a screen that is 3 feet by 7 feet, filling thescreen. What will be the ratio of the area of the slide to its image on the screen?• 1 : 112• 1 : 2304• 2 : 4205• 1 : 12,54418. Find the area of a regular octagon with perimeter 48 cm.•188.1 cm2•190.5 cm2•347.6 cm2•173.8 cm219. Dorothy ran 6 times around a circular track that has a diameter of 47 m. Approximately how far did she run?Use π = 3.14 and round your answer to the nearest meter.•885 m•1328 m•443 m•1734 m20. Find the area.•10.26 cm2•61.56 cm21. Find the volume of the cylinder in terms of π.•24π in.3•48π in.3•56π in.3•288π in.32. Find the volume of the cylinder in terms of π.•287π in.3•275π in.2•275π in.3•287π in.23. A sphere has a volume of 288π ft3. Find the surface area of the sphere.•864π ft2•48π ft2•144π ft2•96π ft24. The volumes of two similar solids are 2197 m3 and 64 m3. The surface area of the larger one is 845 m2. Whatis the surface area of the smaller one?•64 m2•320 m2•80 m2•none of these5. Use a net to find the surface area of the prism.•114 m2•240 m2•290 m2•145 m26. Find the surface area of a sphere that has a diameter of 4 cm.•64π cm2•π cm3•4π cm2•16π cm27. Find the lateral area and the surface area of the cone. Use 3.14 for π and round the answer to the nearesthundredth. The diagram is not to scale.•lateral area: 733.33 ft2; surface area: 690.80 ft2•lateral area: 6908.00 ft2; surface area: 1004.80 ft2•lateral area: 690.80 ft2; surface area: 1004.80 ft2•none of these8. If the ratio of the radii of two spheres is 7 : 2, what is the ratio of the surface areas of the twospheres?•7 : 2•7r2π : 2r2π•49 : 4•343 : 89. Use a net to find the surface area of the prism.•465 m2•720 m2•918 m2•930 m210. Find the height of the cylinder to the nearest tenth of an inch.•94.6 in.•96.6 in.• 4.3 in.• 4.1 in.11. Use formulas to find the lateral area and the surface area of the prism. Show your answer to the nearesthundredth.•63.00 m2; 567.00 m2•36.00 m2; 1134.00 m2•479.22 m2; 533.22 m2•542.22 m2; 596.22 m212. Find the volume of the prism.•942 m3•38 m3•945 m3•1890 m313. The volumes of two similar solids are 1331 m3 and 343 m3. The surface area of the larger one is 484 m2.What is the surface area of the smaller one?•343 m2•1372 m2•196 m2•none of these14. Find the surface area of the sphere.•648π m2•72π m2•324π m2•1296π m215. Find the volume of the prism.•40.5 m3•162 m3•9 m3•81 m316. Find the surface area of the solid. Round to the nearest square foot.•36 ft2•32 ft217. Use formulas to find the surface area of the prism. Show your answer to the nearest hundredth.•75.42 cm2•170.16 cm2•86.94 cm2•69.52 cm218. Which figure is a net for a cube?••••19. Cylinder A has radius 1 and height 4 and cylinder B has radius 2 and height 4. Find the ratio of thevolumes of the two cylinders.• 1 : 4• 5 : 620. Neil had a job helping a jeweler. He had the assignment of counting the faces, vertices, and edges on theemeralds. On the first emerald, Neil counted 9 faces and 16 edges. He quickly realized he didn't have to count the vertices. How many vertices were there?•10 vertices•7 vertices•8 vertices•9 vertices1. Find the value of x if AB = 20, BC = 12, and CD = 13. (not drawn to scale)•18.8•16.5•13.4•14.92. Find the measure of each variable if m∠A = 22 and m = 97. (not drawn to scale)•53; 210•53; 105•75; 210•75; 1053. In the plane of lines X and Y, what is the locus of points equidistant from lines X and Y?•line A•line D•line B•line C4. A small messenger company can only deliver within a certain distance from the company. On the graph below,the circular region represents that part of the city where the company delivers, and the center of the circle represents the location of the company. Which equation represents the boundary for the region where the company delivers?•(x + 3)2 + (y– 1)2 = 49•(x + 3)2 + (y– 3)2 = 98•(x + 1)2 + (y– 3)2 = 98•(x + 3)2 + (y– 3)2 = 495. A low-watt radio station can be heard only within a certain distance from the station. On the graph below,the circular region represents that part of the city where the station can be heard, and the center of the circle represents the location of the station. Which equation represents the boundary for the region where the station can be heard?•(x– 4)2 + (y– 5)2 = 50•(x + 5)2 + (y– 5)2 = 59•(x + 5)2 + (y + 4)2 = 25•(x + 5)2 + (y– 5)2 = 256. Find the center and radius of (x– 8)2 + (y + 7)2 = 64.•(–8, 7); 8•(–7, 8); 64•(8, –7); 8•(–7, –8); 87. is tangent to circle O at B. How close to the circle is point A? (The diagram is not to scale.)• 3• 4.5• 6•7.58. Compare the quantity in Column A with the quantity in Column B.•The quantity in Column A is greater.•The quantity in Column B is greater.•The two quantities are equal.•The relationship cannot be determined from the information given.9. Solve for x.•22•710. Find the value of x.•14.6•8.1•9.4•13.411. Write the standard equation for the circle with center (14, –48) that passes through (0, 0).•(x– 14)2 + (y + 48)2 = 2500•(x + 14)2 + (y– 48)2 = 2500•(x + 14)2 + (y– 48)2 = 50•(x– 14)2 + (y + 48)2 = 5012. Find the measure of ∠BAC.•30°•150°13. Find the value of x.•79•39•99•15914. In space, which description fits the locus of points 3 cm from ?•an open cylinder of diameter 6 cm•an open cylinder of radius 3 cm and two hemispheres of diameter 6 cm each•an open cylinder of radius 3 cm and height 6 cm•an open cylinder of diameter 6 cm and two spheres of radius 3 cm each15. and are tangent to circle O and bisects ∠BPA. If m∠AOC= 68°, how much greater is m∠BCOthan m∠OAD? (The diagram is not to scale.)•22°•112°16. Write the standard equation for the circle with center (6, –8) that passes through (0, 0).•(x + 6)2 + (y– 8)2 = 0•(x– 6)2 + (y + 8)2 = 0•(x + 6)2 + (y– 8)2 = 100•(x– 6)2 + (y + 8)2 = 10017. Find the center and radius of (x + 3)2 + (y + 8)2 = 169.•(3, 8); 13•(–8, 3); 13•(–3, –8); 13•(–8, –3); 16918. , , and are all tangent to circle O. If JA = 8, AL = 13, and CK = 11, what is the perimeter ofΔJKL? (The diagram is not to scale.)•32•64•45•5319. If m = 38, what is m∠YAC?•142°•71°•109°•52°20. Write the standard equation for the circle with center (–16, 30) that passes through (0, 0).•(x + 16)2 + (y– 30)2 = 34•(x + 16)2 + (y– 30)2 = 1156•(x– 16)2 + (y + 30)2 = 1156•(x– 16)2 + (y + 30)2 = 34。
纯英文数学试卷初中答案

Section 1: Multiple Choice (40 points)1. Solve for x: 3x + 5 = 19a) x = 2b) x = 4c) x = 6d) x = 8Answer: b) x = 42. Simplify: 8(3 - 2x) + 4xa) 24 - 16x + 4xb) 24 - 16x + 8xc) 24 - 4x + 4xd) 24 - 8x + 4xAnswer: b) 24 - 16x + 8x3. Solve for y: 2y - 5 = 15a) y = 10b) y = 20c) y = 5d) y = 25Answer: b) y = 204. Factorize: x^2 - 5x + 6a) (x - 2)(x - 3)b) (x + 2)(x + 3)c) (x - 2)(x + 3)Answer: a) (x - 2)(x - 3)5. Find the area of a rectangle with length 12 units and width 5 units.a) 60 square unitsb) 30 square unitsc) 20 square unitsd) 40 square unitsAnswer: a) 60 square units6. Simplify: (3/4) - (2/3)a) 1/12b) 5/12c) 7/12d) 11/12Answer: a) 1/127. Solve for z: 3z + 7 = 2z + 13a) z = 6b) z = 7c) z = 8d) z = 9Answer: a) z = 68. Factorize: 4x^2 - 4x - 15a) (4x + 5)(x - 3)b) (4x - 5)(x + 3)d) (4x + 5)(x + 3)Answer: c) (4x - 5)(x - 3)9. Find the perimeter of a triangle with sides 3 units, 4 units, and 5 units.a) 12 unitsb) 10 unitsc) 11 unitsd) 13 unitsAnswer: d) 13 units10. Solve for m: 5m - 2 = 3m + 10a) m = 4b) m = 6c) m = 8d) m = 10Answer: b) m = 6Section 2: Short Answer (60 points)11. Write the equation of a line with a slope of 2 and passing through the point (1, 3).Answer: y = 2x + 112. Solve the following system of equations:2x + 3y = 8x - y = 1Answer: x = 3, y = 213. Simplify the following expression: (5a - 3b) + (2a + 4b) - (a - 5b)Answer: 6a + 2b14. Find the volume of a cube with side length 5 units.Answer: 125 cubic units15. Solve the following quadratic equation: x^2 - 6x + 9 = 0Answer: x = 316. Find the mean of the following set of numbers: 7, 9, 10, 12, 14, 16, 18Answer: 1217. Simplify the following expression: (3/4) (2/3) + (1/2) (1/4)Answer: 1/218. Find the length of the hypotenuse of a right triangle with legs 6 units and 8 units.Answer: 10 units19. Solve the following equation for x: 4x - 5 = 3x + 2Answer: x = 720. Write the equation of a circle with center at (2, 3) and radius 4 units.Answer: (x - 2)^2 + (y - 3)^2 = 16Section 3: Essay (100 points)21. Explain the difference between a linear equation and a quadratic equation. Provide examples of each and explain how to determine whether a given equation is linear or quadratic.Answer: A linear equation is a first-degree polynomial equation in which each term is either a constant or the product of a constant and a single variable raised to the first power. Examples include y = 2x + 3and 3x - 4y = 12. A quadratic equation is a second-degree polynomial equation in which each term is either a constant, the product of a constant and a single variable raised to the second power, or the product of two variables raised to the first power. Examples include x^2 - 6x + 9 = 0 and y = x^2 - 4. To determine whether a given equation is linear or quadratic, check the highest degree of the variable. If the highest degree is 1, it is linear; if the highest degree is 2, it is quadratic.Total Score: 200 points。
2007加拿大bc省考

English 10 – 0708 Form A
1. What does the first stanza suggest about the speaker? A. He views life as a series of insurmountable obstacles. B. He has come to appreciate the solitude of his quiet existence. C. He is relieved to have made it successfully back to the valley. D. He believes he has faced the biggest challenge he will ever face.
English 10 – 0708 Form A
Page 3
In the following poem, a mountain climber speaks about his life after climbing Mount Everest.
Page 4
Here in Katmandu
by Donald Justice
• Read the short context statement before each passage for useful information. • The numbers in the left margin next to passages tell you where to find information.
加拿大数学10年级练习第二部分

1. Solve – + = 4. Check your solution.•5, –2• 5•6, –1• 62. Solve = . Check your solution.•–•8• 4•no solution3. Divide ÷ .••••4. Solve –= 1. Check your solution.•0•–7•–7, 0•6, –75. The graph of the function y = is on the left. The graph on the right is the same graph translated right threeunits and up three units. What is the equation of the graph on the right?•y = + 3•y = – 3•y = + 3•y = – 36. Divide (11x2 + 2x3 + 14 + 17x) ÷ (2x + 5).•x2 + 3x + 2 +•x2 + 3x + 1 +•x2– 3x– 1 +•x2– 3x– 2 +7. If no digit appears more than once, how many 2-digit numbers can be formed from the digits 2, 3, 5, 6?• 6• 4•12•658. Divide ÷ (x– 2).•••9. Divide (–3x3 + 4x–5) ÷ (x + 3).•–3x2 + 9x– 23 +•–3x2 + 13x + 39 –•–3x2 + 9x + 31 –•–3x2 + 13x– 44 +10. Divide ÷ .••••11. Multiply • .••••12. Simplify .•••13. Divide (6x2– 5x+ 2) ÷ (3x + 2).•2x + 3•2x– 3 –•2x + 3 –•2x– 3 +14. Simplify .•x2– 9••–x– 5•x + 515. Jamestown Builders has a development of new homes. There are six different floor plans, five exterior colors,and an option of either a two-car or a three-car garage. How many choices are there for one home?•30•90•60•5216. Divide ÷ (x + 6).••••17. Simplify .•••18. The rate of discount R, can be determined using the formula R = 1 –, where P is the regular price ofan item and D is the discount, or amount saved off the regular price. Find the rate of discount on a sweater witha regular price of $28.99 that is on sale for $10.99.•37.9%•$10.99•$18•62.1%19. Fifteen students volunteer for a committee. How many different eight-person committees can be chosen?•6435 committees•40,320 committees•5040 committees•259,459,200 committees20. Divide (2x2 + 4x3– 9 – 10x) ÷ (2x + 3).•2x2– 2x– 1 –•2x2 + 2x + 2 –•2x2 + 2x + 1 –•2x2– 2x– 2 –1. Identify the graph of 2x– 5y = –10.••••2. Write an equation for the translation of y = |x| left 0.3 units.•y = |x| – 0.3•y = |x| + 0.3•y = |x– 0.3|•y = |x + 0.3|3. Which is the graph of y = |x + 4| – 2?••••4. The rate of change is constant in the graph. Find the rate of change. Explain what the rate of change means forthe situation.•–200, value drops $200 every year•–, value drops $200 every 3 years•–20, value drops $20 every year•–60, value drops $60 every year5. Identify the graph of y = –|x– 5| by translating y = –|x|.••••6. Identify the graph of –10x + 5y = –50.••••7. A line passes through (–7, 1) and (4, 4). Identify the point-slope and slope-intercept form of an equation ofthe line.•point-slope: y– 1 = (x + 7); slope-intercept: y = x +•point-slope: y + 7 = (x– 1); slope-intercept: y = x–•point-slope: y– 1 = (x + 7); slope-intercept: y = x +•point-slope: y + 7 = (x– 1); slope-intercept: y = x–8. As water is poured into a tank, the volume was measured every minute. It produced the graph below. What was thevolume at three minutes?• 3.0• 6.0• 4.0•7.99. The table shows the time spent researching the stock market each week and the average weekly percent gain foran investor over one year. Use a trend line to predict the average weekly percent gain from researching the stock market for 20 hours per week.•about 10%•about 20%•about 0.5%•about 8%10. Write an equation for the translation of y = |x| down 0.75 units.•y = |x+ 0.75|•y = |x| + 0.75•y = |x| – 0.75•y = |x– 0.75|11. Identify the graph of y = |x| + 3.5 by translating y = |x|.••••12. Which is the graph of y = |x + 5| + 3?••••13. Graph the data for the times Shauna spent learning music for the violin and her scores at the annual solocompetitions. Find an equation of the trend line of the data.•y– 31 = 2.5(x– 10)•x– 31 = 2.5(y– 10)•y– 10 = 2.5(x– 21)•y + 31 = 2.5(x + 10)14. Find the slope of the line.• 6•–6••–15. Find the slope of the line.•–1• 5•–5•undefined16. Write the slope-intercept form of the equation for the line.•y = –x + 7•y = x– 7•y = –x– 7•y = x + 717. Find the slope of a line perpendicular to the graph of 5x + y = 7.•–5••–• 518. Describe the relationship between the average June temperatures and the latitude positions of cities on thescatter plot below.•The temperatures are about the same at all latitudes.•The lower the latitude, the lower the temperature.•The higher the latitude, the lower the temperature.•The higher the latitude, the higher the temperature.19. Find the slope of the line.•–•–3•0•undefined20. The rate of change is constant in the graph. Find the rate of change. Explain what the rate of change meansfor the situation.•–20, value drops $20 every year•–60, value drops $60 every year•–, value drops $200 every 3 years•–200, value drops $200 every year1. You decide to market your own custom computer software. You must invest $4305 on computer hardware, and spend$2.75 to buy and package each disk. If each program sells for $13.00, how many copies must you sell to break even?•421 copies•274 copies•273 copies•420 copies2. Solve by elimination.3x– 4y = 9–3x + 2y = 9•(–27, –9)•(–9, –9)•(–3, –6)•(3, 9)3. You decide to market your own custom computer software. You must invest $3255 on computer hardware, and spend$2.90 to buy and package each disk. If each program sells for $13.75, how many copies must you sell to break even?•195 copies•301 copies•196 copies•300 copies4. What is the graph of y > –6x + 3?••••5. Which ordered pair is a solution of the system?7x + 2y = –39–8x + 3y = –3•(–3, –9)•(–7, –11)•(–4, –7)•(–1, –10)6. Solve by elimination.4x–y = 43x + y = 10•(0, –4)•(12, 4)•(2, 4)•(4, 12)7. The length of a rectangle is 3 centimeters more than 3 times the width. If the perimeter of the rectangle is46 centimeters, what are the dimensions of the rectangle?•length = 5 cm; width = 18 cm•length = 13 cm; width = 5 cm•length = 13 cm; width = 8 cm•length = 18 cm; width = 5 cm8. Which point is a solution of y≥ 4x– 5?•(1, 1)•(2, 1)•(3, 0)•(3, 4)9. Which system has infinitely many solutions?•x + y = 3x + y = 4•2x + y = 54x + 2y = 10•2x + y = 74x– 2y = 14•3x–y = 2x– 3y = –210. Solve the system using substitution.f(x) = x– 4f(x) = x– 6•(3, –3)•(3, –4)•(6, –2)•(4, 6)11. A motorboat can go 16 miles downstream on a river in 20 minutes. It takes 30 minutes for this boat to go backupstream the same 16 miles. Find the speed of the boat in still water.•40 mi/h•32 mi/h•8 mi/h•48 mi/h12. Which system has no solution?•4x– 2y = 1y = 2x– 7•y = 2x + 2x– 2y = 1•y = –x + 1x–y = 1•3x–y = 3y = –3x + 313. A motorboat can go 8 miles downstream on a river in 20 minutes. It takes 30 minutes for this boat to go backupstream the same 8 miles. Find the speed of the current.•20 mi/h•16 mi/h•24 mi/h• 4 mi/h14. Find the solution of the system.3y = –x + 2y = –x + 9•(3, 6)•(20, –4)•(10, –1)•(–1, 8)15. Mrs. Huang operates a soybean farm outside Grinnell, Iowa. To keep her operating costs down, she buys many productsin bulk and transfers them to smaller containers for use on the farm. Often the bulk products are not the correct concentration and need to be custom mixed before Mrs. Huang can use them. One day she wants to apply herbicide toa large field. A solution of 67% herbicide is to be mixed with a solution of 46% herbicide to form 42 liters ofa 55% solution. How much of the 67% solution must she use?•34 L•23 L•18 L•35 L16. What is the graph of y≤ 2x– 2?••••17. Write x– 2y < –2 in slope-intercept form. Then graph the inequality.•y > x + 1•y < x + 1•y < –x + 1•y > –x + 118. Which graphing calculator screen shows the solution of the system?y = –x + 3y = 6x– 5••••19. Identify the graph of y≤ 3x– 3.••••20. Mike and Kim invest $10,000 in equipment to print yearbooks for schools. Each yearbook costs $5.75 to printand sells for $25. How many yearbooks must they sell before their business breaks even?•500 yearbooks•520 yearbooks•400 yearbooks•1,740 yearbooks1. This graph represents a translation of the graph of y = |x|. What is the equation of this graph?•y = |x− 2|•y = |x| − 2•y= |x + 2|•y = |x| + 22. Find an equation for the linear model of the situation below and use it to make a prediction. A train is travelingnorth at a constant rate. At 3:00 P.M. it is 55 miles north of a city. At 4:15 P.M. it is 80 miles north of the city.If d represents the distance in miles, and t represents the time in hours, how many miles north of the city will the train be at 5:45 P.M.?•d = 64t + 55; d = 231 miles•d = 80t + 55; d = 255 miles•d = 64t + 55; d = 96 miles•d = 100t + 55; d = 285 miles3. What are the domain and range of this function?•Domain: x > 0; Range: y > 0•Domain: x≥ 0; Range: y≥ 0•Domain: all real numbers; Range: all real numbers•Domain: positive integers; Range: positive integers4. What is the graph of y > |x− 3|?••••5. An electronics store makes a profit of $55 for every standard CD player sold and $77 for every portable CD playersold. The manager's goal is to make at least $385 a day on sales of both standard and portable CD players. If s represents the number of standard CD players sold, and p represents the number of portable CD players sold, which inequality and graph represent the manager's daily goal?•55s + 77p≤ 385•77s + 55p≥ 385•55s + 77p≥ 385•77s + 55p≤ 3856. What is an equation of the inequality?•y≤ |x− 1| − 2•y≥ |x + 1| − 2•y≥ |x− 1| − 27. What is the graph of y≥ |x + 1| − 1?••••8. What is the graph of y = −|3x|?•••9. What is the graph of y = |−x− 4|?•••10. What is the equation of y = |x| translated 1 unit up and 6 units to the left?•y = |x− 6| + 1•y = |x− 1| + 6•y = |x + 1| + 6•y = |x + 6| + 111. What is the graph of y = |x| − |2x|?••••not here12. The scatter plot below shows the weights in ounces of several kittens at various ages. What is the best equationof the trend line through these points?•y = 10x•y = 4x•y = x + 10•y = 4x + 1013. What is the standard form of the equation of the line whose slope is −2 and which contains the point (3, −5)?•2x + y = 1•2x + y = −8•2x + y = 11•2x + y = −714. Find f(−5) if f(x) = −8x− 1.•39•−41•−39•1215. The points in the table have been graphed in the scatter plot below. What is the best equation for the trendline, and the value of y when x is 16?•y = 1.25x + 1; y = 21•y = −1.25x + 1; y = 19•y = 2x + 1; y = 33•y = 0.8x + 1; y = 11.816. A linear model for the situation below passes through the origin.A manufacturer can produce 4200 gears in 8 hours. How many gears can be made in 1 hour?•525•262.5•1050•60017. What is an inequality for this graph?•y− 3x > −5•y− 3x≥ −5•y− 3x≤ −5•y− 3x < −518. Which set of points represents the graph of the function below?(−3, 2), (−1, −1), (2, 2), (4, 0)••••19. What is the graph of the line whose slope is and whose y-intercept is 4?••••20. The graph below models the burning of a candle. At time t = 0, the candle was 8 inches tall. If the candle burnsat a rate of 2 inches per hour, what equation models the height h of the candle after burning for t hours?•h = 2t + 8•h = 8t + 2•h = 8t− 2•h = −2t + 81. Which system of equations is the result of using the elimination method to solve the system ,and what is the solution?•; (1, 12)•; (11, 13)•; (2, )•; (13, 4)2. Which point lies in the plane represented by the equation 2x + 7y + 3z = 25?•(−6, −4, 5)•(3, −3, 3)•(−3, 3, −2)•(6, 1, −3)3. The equation P= 5x+ 3y represents the objective function for this linear programming model. What is the vertexof the feasible region shown below that results in the maximum value of P?•(12, 14)•(12, 2)•(14, 4)•(8, 12)4. Use elimination to find the solution of the system .•(−1, , 3)•(−1, 3, 2)•(1, 3, 0)•(−1, 2, 4)5. Find the values of x and y that maximize the objective function for this graph.Maximum for P = 3x + 2y•(0, 9)•(0, 0)•(5, 0)•(1, 8)6. Which system is inconsistent?••••7. A point (x, y, z) can be used to represent the location of a geographical point in space, where x representsthe latitude in degrees, y represents the longitude in degrees, and z represents the elevation in feet above or below sea level.Jerimoth Hill is the highest point of elevation in Rhode Island. It is 812 feet above sea level and lies at about 42° north latitude and 72° west longitude. What ordered triple represents the location of Jerimoth Hill?•(42°, −72°, −812)•(42°, −72°, 812)•(−72°, 42°, 812)•(−72°, 42°, −812)8. What is the graph of −3x + 5y +10z = 15?••••9. What steps would you use to plot (−3, −4, 0) in a three-dimensional coordinate system?•From the origin, move back 3 units and down 4 units.•From the origin, move back 3 units and left 4 units.•From the origin, move down 3 units and left 4 units.•From the origin, move back 3 units and up 4 units.10. Identify the graph of the system and its solution.•(3, 0)•(−3, 0)•infinite number of solutions•no solution11. What is the solution of this system?•(, )•(−2, −3)•(−14, −23)•(−3, −2)12. A small fish market sells only tuna and salmon. A tuna costs the fish market $0.75 per pound to buy and $2.53per pound to clean and package. A salmon costs the fish market $3.00 per pound to buy and $2.75 per pound to clean and package. The fish market makes $2.50 per pound profit for each tuna it sells and $2.80 per pound profit for each salmon it sells. The fish market owner can spend only $159.00 per day to buy fish and $197.34 per day to clean and package the fish.The graph below represents the feasible region for this linear programming model. What are the coordinates of the vertices of the feasible region, and what are the vales of t and s that maximize the objective function?; P = 2.50t + 2.80s•(0, 0), (0, 53), (71.76, 0), (46, 28); t = 46 and s = 28.•(0, 0), (53, 0), (0, 71.76), (28, 46); t = 28 and s = 46.•(0, 0), (0, 53), (71.76, 0), (28, 46); t = 28 and s = 46.•(0, 0), (0, 53), (71.76, 0), (46, 28); t = 0 and s = 53.13. Identify the graph of the system and its solution.•(−5, −4)•(, −)•(, −)•(−1, 8)14. Identify the graph that shows the solution to the system of inequalities.••••15. What is the maximum for the objective function for this graph?Maximum for P = 5x + 2y•90•80•250•56016. How could you solve this system using substitution?•Substitute for r in the equation t = 2r + 3.•Substitute for t in the equation t = 2r + 3.•Substitute 2r + 3 for t in the equation 5r− 4t = 6.•Substitute 2r + 3 for r in the equation 5r− 4t = 6.17. How could you solve this system by substitution?•Substitute 6 for c in the equation 3c + 5d = 2.•Substitute 6 for d into the equation 3c + 5d = 2.•Substitute for d into the equation d = 6.•Substitute 3(3c + 5d) for 6 in the equation d = 6.18. Identify the graph that shows the solution to the system of inequalities.••••19. Identify the graph that shows the solution to the system of inequalities.••••20. Locate the point (4, −3, 3) in a three-dimensional coordinate system.••••none of these。
加拿大数学10年级练习第四部分几何

1. Find the ratio of the perimeter of the larger rectangle to the perimeter of the smaller rectangle.∙∙∙∙2. Find the area of the triangle with ∠A = 41, b = 5 ft, and c = 4 ft. Round your answer to two decimal places. ∙ 6.56 ft2∙10.00 ft2∙7.55 ft2∙13.12 ft23. Find the area of kite ABCD if BD = 48 cm, AB = 25 cm, and BC = 26. The kite is not drawn to scale.∙289 cm2∙70 cm2∙816 cm2∙408 cm24. The diameter of a basketball rim is 18 inches. A standard basketball has a circumference of 30 inches. Abouthow much room is there between the ball and the rim in a shot in which the ball goes in exactly in the center of the rim?∙none of these5. Find the area.∙718 square units∙545 square units∙534.5 square units∙701 square units6. Name the major arc and find its measure.∙m = 275∙m = 170∙m = 85∙m = 2757. Find the area of the regular polygon. Round your answer to the nearest tenth.∙110.2 in.28. Name the minor arc and find its measure.∙m = 275∙m = 85∙m = 170∙m = 2759. Find the area of ΔABC. The figure is not drawn to scale.∙26.06 cm2∙28.00 cm2∙22.73 cm2∙24.95 cm210. Find the circumference of the circle. Use as an approximation of π.∙11 cm∙5 cm∙6 cm11. If a dart hits the target at random, what it the probability that it will land in the unshaded region?∙∙∙∙12. Find the area of the circle. Use π = 3.14 and round to the nearest hundredth.∙91.56 m2∙16.96 m2∙ 5.72 m2∙22.89 m213. Find the area of a regular pentagon with side 6 cm.∙45.2 cm2∙123.9 cm214. Find the probability that an object falling randomly on the figure will land in the shaded area.∙0.32∙0.36∙0.5∙0.2615. Find the area.∙102.9 yd2∙205.8 yd2∙35.35 yd2∙105.5 yd216. Two concentric circles have radii of 14 cm and 24 cm. Find the probability to the nearest thousandth that apoint chosen at random from the circles is located outside the smaller circle and inside the larger one.∙0.066∙0.58317. A slide that is inches by inches is projected onto a screen that is 3 feet by 7 feet, filling the screen.What will be the ratio of the area of the slide to its image on the screen?∙ 1 : 112∙ 1 : 2304∙ 2 : 4205∙ 1 : 12,54418. Find the area of a regular octagon with perimeter 48 cm.∙188.1 cm2∙190.5 cm2∙347.6 cm2∙173.8 cm219. Dorothy ran 6 times around a circular track that has a diameter of 47 m. Approximately how far did she run?Use π = 3.14 and round your answer to the nearest meter.∙885 m∙1328 m∙443 m∙1734 m20. Find the area.∙10.26 cm21. Find the volume of the cylinder in terms of π.∙24π in.3∙48π in.3∙56π in.3∙288π in.32. Find the volume of the cylinder in terms of π.∙287π in.3∙275π in.2∙275π in.3∙287π in.23. A sphere has a volume of 288π ft3. Find the surface area of the sphere.∙864π ft2∙48π ft2∙144π ft2∙96π ft24. The volumes of two similar solids are 2197 m3 and 64 m3. The surface area of the larger one is 845 m2. What is∙64 m2∙320 m2∙80 m2∙none of these5. Use a net to find the surface area of the prism.∙114 m2∙240 m2∙290 m2∙145 m26. Find the surface area of a sphere that has a diameter of 4 cm.∙64π cm2∙π cm3∙4π cm2∙16π cm27. Find the lateral area and the surface area of the cone. Use 3.14 for πand round the answer to the nearest hundredth.The diagram is not to scale.∙lateral area: 733.33 ft2; surface area: 690.80 ft2∙lateral area: 690.80 ft2; surface area: 1004.80 ft2∙none of these8. If the ratio of the radii of two spheres is 7 : 2, what is the ratio of the surface areas of the two spheres? ∙7 : 2∙7r2π : 2r2π∙49 : 4∙343 : 89. Use a net to find the surface area of the prism.∙465 m2∙720 m2∙918 m2∙930 m210. Find the height of the cylinder to the nearest tenth of an inch.∙94.6 in.∙96.6 in.∙ 4.3 in.∙ 4.1 in.11. Use formulas to find the lateral area and the surface area of the prism. Show your answer to the nearest hundredth.∙63.00 m2; 567.00 m2∙36.00 m2; 1134.00 m2∙479.22 m2; 533.22 m2∙542.22 m2; 596.22 m212. Find the volume of the prism.∙942 m3∙38 m3∙945 m3∙1890 m313. The volumes of two similar solids are 1331 m3 and 343 m3. The surface area of the larger one is 484 m2. Whatis the surface area of the smaller one?∙343 m2∙1372 m2∙196 m2∙none of these14. Find the surface area of the sphere.∙648π m2∙72π m2∙324π m2∙1296π m215. Find the volume of the prism.∙40.5 m3∙162 m3∙9 m3∙81 m316. Find the surface area of the solid. Round to the nearest square foot.∙36 ft2∙68 ft217. Use formulas to find the surface area of the prism. Show your answer to the nearest hundredth.∙75.42 cm2∙170.16 cm2∙86.94 cm2∙69.52 cm218. Which figure is a net for a cube?∙∙∙∙19. Cylinder A has radius 1 and height 4 and cylinder B has radius 2 and height 4. Find the ratio of the volumesof the two cylinders.∙ 1 : 4∙ 1 : 120. Neil had a job helping a jeweler. He had the assignment of counting the faces, vertices, and edges on the emeralds.On the first emerald, Neil counted 9 faces and 16 edges. He quickly realized he didn't have to count the vertices.How many vertices were there?∙10 vertices∙7 vertices∙8 vertices∙9 vertices1. Find the value of x if AB = 20, BC = 12, and CD = 13. (not drawn to scale)∙18.8∙16.5∙13.4∙14.92. Find the measure of each variable if m∠A = 22 and m = 97. (not drawn to scale)∙53; 210∙53; 105∙75; 210∙75; 1053. In the plane of lines X and Y, what is the locus of points equidistant from lines X and Y?∙line A∙line D∙line B∙line C4. A small messenger company can only deliver within a certain distance from the company. On the graph below, thecircular region represents that part of the city where the company delivers, and the center of the circle represents the location of the company. Which equation represents the boundary for the region where the company delivers?∙(x + 3)2 + (y– 1)2 = 49∙(x + 3)2 + (y– 3)2 = 98∙(x + 1)2 + (y– 3)2 = 98∙(x + 3)2 + (y– 3)2 = 495. A low-watt radio station can be heard only within a certain distance from the station. On the graph below, thecircular region represents that part of the city where the station can be heard, and the center of the circle represents the location of the station. Which equation represents the boundary for the region where the station can be heard?∙(x– 4)2 + (y– 5)2 = 50∙(x + 5)2 + (y– 5)2 = 59∙(x + 5)2 + (y + 4)2 = 25∙(x + 5)2 + (y– 5)2 = 256. Find the center and radius of (x– 8)2 + (y + 7)2 = 64.∙(–8, 7); 8∙(–7, 8); 64∙(8, –7); 8∙(–7, –8); 87. is tangent to circle O at B. How close to the circle is point A? (The diagram is not to scale.)∙ 3∙ 4.5∙ 6∙7.58. Compare the quantity in Column A with the quantity in Column B.∙The quantity in Column A is greater.∙The quantity in Column B is greater.∙The two quantities are equal.∙The relationship cannot be determined from the information given.9. Solve for x.∙22∙710. Find the value of x.∙14.6∙8.1∙9.4∙13.411. Write the standard equation for the circle with center (14, –48) that passes through (0, 0). ∙(x– 14)2 + (y + 48)2 = 2500∙(x + 14)2 + (y– 48)2 = 2500∙(x + 14)2 + (y– 48)2 = 50∙(x– 14)2 + (y + 48)2 = 5012. Find the measure of ∠BAC.∙30°∙150°13. Find the value of x.∙79∙39∙99∙15914. In space, which description fits the locus of points 3 cm from ?∙an open cylinder of diameter 6 cm∙an open cylinder of radius 3 cm and two hemispheres of diameter 6 cm each∙an open cylinder of radius 3 cm and height 6 cm∙an open cylinder of diameter 6 cm and two spheres of radius 3 cm each15. and are tangent to circle O and bisects ∠BPA. If m∠AOC= 68°, how much greater is m∠BCOthan m∠OAD? (The diagram is not to scale.)∙22°∙112°16. Write the standard equation for the circle with center (6, –8) that passes through (0, 0).∙(x + 6)2 + (y– 8)2 = 0∙(x– 6)2 + (y + 8)2 = 0∙(x + 6)2 + (y– 8)2 = 100∙(x– 6)2 + (y + 8)2 = 10017. Find the center and radius of (x + 3)2 + (y + 8)2 = 169.∙(3, 8); 13∙(–8, 3); 13∙(–3, –8); 13∙(–8, –3); 16918. , , and are all tangent to circle O. If JA= 8, AL= 13, and CK= 11, what is the perimeter of ΔJKL?(The diagram is not to scale.)∙32∙64∙45∙5319. If m = 38, what is m∠YAC?∙109°∙52°20. Write the standard equation for the circle with center (–16, 30) that passes through (0, 0). ∙(x + 16)2 + (y– 30)2 = 34∙(x + 16)2 + (y– 30)2 = 1156∙(x– 16)2 + (y + 30)2 = 1156∙(x– 16)2 + (y + 30)2 = 34。
加拿大数学10年级练习第一部分几何
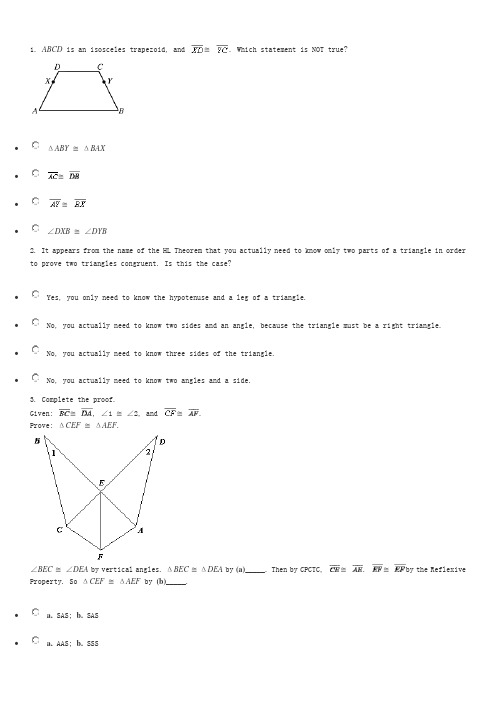
1. ABCD is an isosceles trapezoid, and ≅. Which statement is NOT true?•ΔABY≅ΔBAX•≅•≅•∠DXB≅∠DYB2. It appears from the name of the HL Theorem that you actually need to know only two parts of a triangle in orderto prove two triangles congruent. Is this the case?•Yes, you only need to know the hypotenuse and a leg of a triangle.•No, you actually need to know two sides and an angle, because the triangle must be a right triangle.•No, you actually need to know three sides of the triangle.•No, you actually need to know two angles and a side.3. Complete the proof.Given: ≅, ∠1 ≅∠2, and ≅.Prove: ΔCEF≅ΔAEF.∠BEC≅∠DEA by vertical angles. ΔBEC≅ΔDEA by (a)_____. Then by CPCTC, ≅. ≅by the Reflexive Property. So ΔCEF≅ΔAEF by (b)_____.• a. SAS; b. SAS• a. AAS; b. SSS• a. ASA; b. SSS• a. AAS; b. HL4. Complete the proof.Given: ≅, ∠1 ≅∠2Prove: ΔBEA≅ΔDEC≅and ∠1 ≅∠2, so ΔBCA≅ΔDAC by SAS. Then, since (a)_____, ≅, ≅. ∠BEA≅∠DEC by (b)_____, so ΔBEA≅ΔDEC by (c)_____.• a. CPCTC; b. vertical angles; c. SAS• a. CPCTC; b. vertical angles; c. AAS• a. CPCTC; b. vertical angles; c. SSS• a. SAS; b. vertical angles; c. SSS5. What additional information can be used to prove the triangles congruent by the HL Theorem?•m∠BCE = 90•AB > AC•≅•≅6. Suppose ΔCED≅ΔDBC. If m∠EDC = 63 and m∠DBC = 82, what is m∠DCE?•63•82•145•357. If ∠A≅∠D and ∠C≅∠F, which statement would NOT prove that ΔABC≅ΔDEF?••∠B≅∠E•≅•none of these8. Determine what information you would need to know in order to use the SSS Congruence Postulate to show that thetriangles are congruent.•∠BAD≅∠CDB•≅•∠ADB≅∠CBD•≅9. Suppose ΔBCA≅ΔECD. Which statement is NOT necessarily true?•≅•∠A≅∠D•≅•∠BCA≅∠DCE10. In which triangles could you efficiently prove Δ1 ≅Δ2 using the HL Theorem?•II only•III only•II and III•I only11. Complete the proof.Given: ≅, ∠1 ≅∠2, and ≅.Prove: ΔCEF≅ΔAEF.∠BEC≅∠DEA with vetical angles. ΔBEC≅ΔDEA by (a)_____. Then by (b)_____, ≅. ≅by the Reflexive Property. So ΔCEF≅ΔAEF by (c)_____.• a. AAS; b. CPCTC; c. SSS• a. SAS; b. CPCTC; c. SSS• a. AAS; b. CPCTC; c. SAS• a. SSS; b. CPCTC; c. ASA12. In the paper airplane, ABCD≅EFGH, m∠B = m∠BCD = 90, and m∠BAD = 140. Find m∠GHE.•130•90•40•14013. Find the value of x.•x = –2•x = 9•x = 21•none of these14. Explain how you can use SSS, SAS, ASA, or AAS with CPCTC to prove that ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Symmetric Property, ≅. By SAS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By ASA, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By SAS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By SSS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.15. Complete the proof.Given: bisects ∠URS and bisects ∠UTS.Prove: ΔURT≅ΔSRT.•Reflexive property•definition of angle bisector•HL Theorem•CPCTC16. Complete the proof.Given: ∠RSQ≅∠TSQ, ∠RQS≅∠TQS.Prove: ≅.∠RSQ≅∠TSQ is given, as is ∠RQS≅∠TQS. By the Reflexive Property, ≅.ΔSRQ≅ΔSTQ by (a)_____, so ≅by (b)_____.• a. ASA; b. CPCTC• a. HL; b. CPCTC• a. SSS; b. CPCTC• a. SAS; b. CPCTC17. Determine which triangles are congruent by AAS using the information in the diagram below.•ΔABF≅ΔEDF•ΔADC≅ΔEBC•ΔABE≅ΔEDA•ΔABE≅ΔCBE18. Complete the proof.Given: bisects ∠EBC and bisects ∠ECC.Prove:ΔEBD≅ΔCBD.•Same-Side Interior Angles Theorem•given•SSS postulate•Triangle Inequality Theorem19. ΔABD≅ΔCBD. Name the theorem or postulate that justifies the congruence.•SAS•AAS•ASA•none of these1. Determine whether each quadrilateral can be a parallelogram. If not, write impossible.a. Two adjacent angles are right angles, but the quadrilateral is not a rectangle.b. All of the angles are congruent.• a. impossible; b. parallelogram• a. parallelogram; b. parallelogram• a. parallelogram; b. impossible• a. impossible; b. impossible2. Which statement is true?•All rectangles are squares.•All quadrilaterals are squares.•All quadrilaterals are parallelograms.•All parallelograms are quadrilaterals.3. Which statement can be used to determine whether quadrilateral XYZW must be a parallelogram?•≅and ≅•≅and ≅•≅and ≅•XW = WZ and XY = YZ4. Choose the best name for the parallelogram and find the measures of the numbered angles.•Square; all numbered angles are equal to 45°.•Rhombus; all numbered angles are equal to 115°.•Rhombus; all numbered angles are equal to 25°.•Square; all numbered angles are equal to 50°.5. Given: quadrilateral ABCD with A(–2, 3), B(2, –4), C(9, 0), D(5, 7). Then ABCD is a rectangle because•the slopes of the sides in pairs are negative reciprocals.•the product of the slopes of the diagonals is –1.•the figure has four vertices.•opposite sides have the same slope.6. Given the parallelogram below, find coordinates for P, without using any new variables.•(a–c, b)•(a + c, b)•(c, b)•(c, a)7. Find the values of the variables for the rectangle. Then find the lengths of the sides.•x = 7, y = 5; side lengths: 70, 45•x = 5, y = 7; side lengths: 33, 94•x = 5, y = 7; side lengths: 50, 63•x = 7, y = 5; side lengths: 45, 458. Determine whether the quadrilateral is a parallelogram. Explain.≅and ≅•Yes; if two opposite sides are congruent, then the quadrilateral is a parallelogram.•No; if the diagonals of a quadrilateral bisect each other, this is not enough to prove that the quadrilateral is a parallelogram.•No; if two opposite sides are congruent, this is not enough to prove that the quadrilateral is a parallelogram.•Yes; if the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.9. Can coordinate geometry be used to prove that opposite sides and in quadrilateral EFGH are congruent?•Yes; use the Distance Formula to show that the diagonals are congruent.•No; you can only show that EF is parallel to GH by using coordinate geometry.•Yes; use the Distance Formula between vertices E and F, and between vertices G and H.•No; you can only find the slopes of EF and GH by using coordinate geometry.10. A square WXYZ has the vertices W(b, b), X(b, –b), Y(–b, –b), and Z(–b, b). Which vertex is in QuadrantII?•W•Z•X•Y11. ∠J and ∠M are base angles of isosceles trapezoid JKLM. If m∠J = 21x + 4, m∠K = 12x– 8, and ∠M = 14x+ 10, find the value of x.•• 2•–•–912. Suppose you are using coordinate geometry to prove that quadrilateral WXYZ is a square. Explain why no twosides should be parallel to the y-axis.•The x-axis would intersect two sides of the square, so the coordinates of the corners would not be clear.•Sides which are parallel to the y-axis would have an undefined slope, so you cannot prove numerically that these sides are parallel.•Points on the sides which are parallel to the y-axis could have any y-value.•The sides of the square which are parallel to the y-axis could be easily confused with the y-axis.13. Find AM if PN = 8 and AO = 5.•8• 3•13• 514. Given square ABCD, where A = (0, a), B = (a, a), C = (0, 0), and D = (a, 0). To prove that the diagonal ADis times the length of side CD, first use _____ to find that = a and = a. Therefore, the ratio= , or .•the definition of isosceles triangle ACD•the definition of right angle C•the Distance Formula•the definition of the origin C = (0, 0)15. A farmer is building a fence for his yard. He is considering two designs, which are shown below. Explain whythe quadrilaterals formed by the horizontal rails and the slanting boards are parallelograms in both designs.•The horizontal rails are parallel to each other. A parallelogram has exactly one pair of parallel sides, so both quadrilaterals are parallelograms.•The horizontal rails are congruent to each other. The slanting boards are all congruent to each other. A parallelogram has two pairs of adjacent sides, but opposite sides are not congruent, so both quadrilaterals are parallelograms.•The horizontal rails are congruent to each other. The slanting boards are all congruent to each other. A parallelogram has four congruent sides, so both quadrilaterals are parallelograms.•The horizontal rails are parallel to each other. The identical slanting boards all slant at the same angle, so the sides are parallel. A parallelogram has both pairs of sides parallel, so both quadrilaterals are parallelograms.16. A rhombus is centered on the origin. One side of the rhombus goes through the points (a, 0) and (0, b). Whatare possible coordinates for one of the other sides?•(–a, 0), (a, 0)•(b, 0), (0, –a)•(0, –b), (0, b)•(–a, 0), (0, –b)17. If a quadrilateral is a parallelogram, then its opposite sides are _____.•perpendicular•adjacent•congruent•none of these18. Find the value of each variable in the parallelogram. m∠1 = 10x, m∠2 = x + y, and m∠3 = 18z.•x = 9, y = 81, z = 5•x = 18, y = 167, z = 5•x = 18, y = 162, z = 10•x = 9, y = 86, z = 019. Complete ≅ _____ for parallelogram EFGH. Then state a definition or theorem as the reason.•; because the angles of a parallelogram bisect each other•; because the diagonals of a parallelogram bisect each other•; because the diagonals of a parallelogram bisect each other•; because the angles of a parallelogram bisect each other20. Which of the following sets of points represents a line segment in Quadrant III and its reflection in the x-axis?(Use the positive numbers a, b, c, d for the coordinates of the endpoints).•(a, b), (c, d); reflection (–a, b), (–c, d)•(–a, –b), (–c, –d); reflection (–a, b), (–c, d)•(a, b), (c, d); reflection (a, –b), (c, –d)•(–a, –b), (–c, –d); reflection (a, –b), (c, –d)1. Solve for a and b.•a = , b =•a = , b =•a = , b =•a = , b =2. State whether ΔADB∼ΔCDB, and if so, identify the theorem that proves the triangles similar.•yes, SSS∼•yes, AA∼•yes, SAS∼•no3. ABCDE∼GHJDF. Complete the congruence and proportion statements.a.∠H≅b.=• a.B; b.AE• a.E; b.DC• a.E; b.AE• a.B; b.DC4. Write a similarity statement for the two triangles.•ΔVUT∼ΔWXY•ΔTVU∼ΔWXY•ΔTUV∼ΔWXY•ΔTUV∼ΔWYX5. Find the geometric mean of 48 and 3.•9•25.5•12•166. The extendable ramp shown below is used to move crates of fruit to loading docks of different heights. When thehorizontal distance AB is 4 feet, the height of the loading dock, BC, is 3 feet. What is the height of the loading dock, DE?•7 ft•9 ft•11 ft7. Find the geometric mean of 20 and 5.•10• 4•12.5•258. In movies and television, the ratio of the width of the screen to the height is called the aspect ratio. Televisionscreens usually have an aspect ratio of 4 : 3, while movie screens usually have an aspect ratio of 1.85 : 1. However, if a movie is made for television in "Letterbox" format, it retains the 1.85 : 1 aspect ratio and fills in the top and bottom parts of the screen with black bars. What would be the height of a movie in "Letterbox" format on a television screen that measures 25 inches along its diagonal? (Hint: First find the width and height of the television screen.)•13.51 in.•10.81 in.•15 in.•8.12 in.9. Use the diagram to determine the height of the tree.•264 ft•72 ft•60 ft•80 ft10. The two rectangles are similar.Which is a correct proportion between corresponding sides?•=•=•=11. Use the Side-Splitter Theorem to find x given that || .•18•12•24• 612. Find OM if bisects ∠NLM, LM = 14, NO = 3, and LN = 4. Round your answer to the nearest hundredth, ifnecessary.•12.27•18.67•0.86•10.513. There is a law that the ratio of the width to length for the American flag should be 10 : 19. Which dimensionsare NOT in the correct ratio?•20 by 38 in.•50 by 95 ft•20 by 44 ft•100 by 190 ft14. If one measurement of a golden rectangle is 6.8 inches, which could be the other measurement?•8.418 in.•11.002 in.• 1.618 in.• 5.182 in.15. If one measurement of a golden rectangle is 8.2 inches, which could be the other measurement?•9.818 in.• 6.582 in.• 1.618 in.• 5.068 in.16. Solve = .•20•19•15•2417. Find OM if bisects ∠NLM, LM =15, NO = 5, and LN = 11. Round your answer to the nearest hundredth, ifnecessary.•33• 6.82• 3.67•8.5918. The width of a golden rectangle is 3 m, which is shorter than the length. What is the length?• 1.85 m• 2.32 m• 3.64 m• 4.85 m19. Find and simplify the ratio of the length to the width of the rectangle.••••20. ΔBGH∼ΔSWQ. What are the pairs of corresponding sides?•BG and SQ, BH and SW, GH and WQ•BG and GB, SQ and QS, GH and HG•BG and SW, BH and SQ, GH and WQ•BG and WQ, BH and SW, GH and SQ1. A building near Atlanta, Georgia, is 181 feet tall. On a particular day at noon it casts a 204-foot shadow. Whatis the sun's angle of elevation at that time?•41.6°•27.5°•62.5°•48.4°2. In right triangle ΔABC, sin A = . What is cos A?••••none of these3. Find the ratio for cos x.••••14. Find the value of x to the nearest meter.•46 m•40 m•35 m•36 m5. Compare the quantity in Column A with the quantity in Column B. The diagram may not be drawn to scale.•The quantity in Column A is greater.•The quantity in Column B is greater.•The two quantities are equal.•The relationship cannot be determined on the basis of the information given.6. How many of these triples could be sides of a right triangle: (27, 36, 45), (12, 17, 20), (24, 32, 40), (14,48, 50)?• 4 triples• 3 triples• 2 triples• 1 triple7. Find the ratio for cos x.•••2•8. Find a third number of the Pythagorean triple that includes 72 and 75.•9•21•37•1049. Find the measure of the marked acute angle to the nearest degree.•62°•28°•61°•118°10. In ΔABC, ∠A is a right angle and m∠B = 60. If AB = 20 ft, find BC. If necessary, round your answer tothe nearest tenth.•10 ft.•40 ft•20 ft•ft11. Find the value of the variable to the nearest hundredth.• 5.28 cm•0.32 cm• 3.13 cm• 5.12 cm12. Find the length of the leg of the right triangle. Leave your answer in simplest radical form.•48••288•13. In ΔABC, ∠A is a right angle and m∠B = 45. If AB = 20 ft, find BC.•10 ft•20 ft•40 ft•20 ft14. Which direction bearing is shown?•19° north of east•19° north of west•19° south of east•19° south of west15. Leslie used the diagram to compute the distance from Ferris to Dunlap to Butte. How much shorter is the distancedirectly from Ferris to Butte than the distance Leslie found?•123 mi•87 mi•36 mi•84 mi16. Which vector has a direction of 31° east of north?••••17. Find the value of x to the nearest tenth.•14.4• 6.3•7.8• 3.118. Find the value of x.•3•6•12• 619. Find the value of x to the nearest tenth.•7.8•18.3•33.0•8.920. Find the value of x to the nearest integer when tan x = 1.483.•58•56•55•571. Which type of isometry is the equivalent of two reflections across two vertical lines?•translation•rotation•glide reflection•none of these2. A section of a tessellated plane is shown below. Which types of symmetry does the tessellated plane have?•glide-reflectional symmetry•translational, rotational, and glide-reflectional symmetry•rotational symmetry•translational and reflectional symmetry3. A blueprint for a house has a scale of 1 : 30. A wall in the blueprint is 7 in. What is the length of the actualwall?•210 ft•21 ft•17.5 ft•none of these4. What is the image of the point (4, –2) after a rotation 270° clockwise about the origin?•(–4, 2)•(4, 2)•(–2, 4)•(2, 4)5. Which graph shows a triangle and its reflection image in the x-axis?••••6. Write a rule to describe a reflection over the y-axis.•(x, y) → (–x, y)•(x, y) → (–x, –y)•(x, y) → (x, –y)•(x, y) → (y, x)•reflectional•rotational•rotational and reflectional•none of these8. Find the image of O(0, 0) after two reflections, first in y = 4, and then in x = –7.•(7, –4)•(–14, 8)•(8, –14)•(–4, 7)9. A section of a tessellated plane is shown below. Which types of symmetry does the tessellated plane have?•rotational symmetry•translational and rotational symmetry•reflectional symmetry•glide-reflectional symmetry•rotational•reflectional•rotational and reflectional•none of these11. Describe the translation 7 units to the left, 12 units up using a vector.•)–12, 7*•)12, –7*•)–7, 12*•)7, –12*12. If the figure has rotational symmetry, find the angle of rotation about the center that results in an imagethat matches the original figure.•120°•90°•72°•It has no rotational symmetry.13. Find the glide reflection image of the solid triangle for the translation )–6, –3* and reflection in y = –1.••••14. If a point P(1, –2) is reflected across the line x = 3, what are the coordinates of its reflection image?•(1, –4)•(1, 8)•(–7, –2)•(5, –2)15. Which translation from thin-lined figure to thick-lined figure is given by the vector )6, 6*?••••16. The dotted triangle is a dilation image of the solid triangle. What is the scale factor?•• 2• 3•17. Find the image of C under the translation described by each vector.a.)4, 5*b.)11, –8*• a.A; b.B• a.B; b.A• a.E; b.D• a.D; b.E18. What is the image of the point (–3, 4) after a rotation of 90° counterclockwise about the point (–3, 0)?•(–7, 0)•(1, 0)•(0, –3)•(–3, –4)19. Which letter has rotational symmetry?• E•X•J•T20. Use scalar multiplication to find the image of the quadrilateral for a dilation with center (0, 0) and scalefactor 2. Graph the quadrilateral and its image.••••。
加拿大数学10年级练习第一部分几何

1. ABCD is an isosceles trapezoid, and ≅. Which statement is NOT true?•ΔABY≅ΔBAX•≅•≅•∠DXB≅∠DYB2. It appears from the name of the HL Theorem that you actually need to know only two parts of a triangle in orderto prove two triangles congruent. Is this the case?•Yes, you only need to know the hypotenuse and a leg of a triangle.•No, you actually need to know two sides and an angle, because the triangle must be a right triangle.•No, you actually need to know three sides of the triangle.•No, you actually need to know two angles and a side.3. Complete the proof.Given: ≅, ∠1 ≅∠2, and ≅.Prove: ΔCEF≅ΔAEF.∠BEC≅∠DEA by vertical angles. ΔBEC≅ΔDEA by (a)_____. Then by CPCTC, ≅. ≅by the Reflexive Property. So ΔCEF≅ΔAEF by (b)_____.• a. SAS; b. SAS• a. AAS; b. SSS• a. ASA; b. SSS• a. AAS; b. HL4. Complete the proof.Given: ≅, ∠1 ≅∠2Prove: ΔBEA≅ΔDEC≅and ∠1 ≅∠2, so ΔBCA≅ΔDAC by SAS. Then, since (a)_____, ≅, ≅. ∠BEA≅∠DEC by (b)_____, so ΔBEA≅ΔDEC by (c)_____.• a. CPCTC; b. vertical angles; c. SAS• a. CPCTC; b. vertical angles; c. AAS• a. CPCTC; b. vertical angles; c. SSS• a. SAS; b. vertical angles; c. SSS5. What additional information can be used to prove the triangles congruent by the HL Theorem?•m∠BCE = 90•AB > AC•≅•≅6. Suppose ΔCED≅ΔDBC. If m∠EDC = 63 and m∠DBC = 82, what is m∠DCE?•63•82•145•357. If ∠A≅∠D and ∠C≅∠F, which statement would NOT prove that ΔABC≅ΔDEF?••∠B≅∠E•≅•none of these8. Determine what information you would need to know in order to use the SSS Congruence Postulate to show that thetriangles are congruent.•∠BAD≅∠CDB•≅•∠ADB≅∠CBD•≅9. Suppose ΔBCA≅ΔECD. Which statement is NOT necessarily true?•≅•∠A≅∠D•≅•∠BCA≅∠DCE10. In which triangles could you efficiently prove Δ1 ≅Δ2 using the HL Theorem?•II only•III only•II and III•I only11. Complete the proof.Given: ≅, ∠1 ≅∠2, and ≅.Prove: ΔCEF≅ΔAEF.∠BEC≅∠DEA with vetical angles. ΔBEC≅ΔDEA by (a)_____. Then by (b)_____, ≅. ≅by the Reflexive Property. So ΔCEF≅ΔAEF by (c)_____.• a. AAS; b. CPCTC; c. SSS• a. SAS; b. CPCTC; c. SSS• a. AAS; b. CPCTC; c. SAS• a. SSS; b. CPCTC; c. ASA12. In the paper airplane, ABCD≅EFGH, m∠B = m∠BCD = 90, and m∠BAD = 140. Find m∠GHE.•130•90•40•14013. Find the value of x.•x = –2•x = 9•x = 21•none of these14. Explain how you can use SSS, SAS, ASA, or AAS with CPCTC to prove that ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Symmetric Property, ≅. By SAS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By ASA, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By SAS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By SSS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.15. Complete the proof.Given: bisects ∠URS and bisects ∠UTS.Prove: ΔURT≅ΔSRT.•Reflexive property•definition of angle bisector•HL Theorem•CPCTC16. Complete the proof.Given: ∠RSQ≅∠TSQ, ∠RQS≅∠TQS.Prove: ≅.∠RSQ≅∠TSQ is given, as is ∠RQS≅∠TQS. By the Reflexive Property, ≅.ΔSRQ≅ΔSTQ by (a)_____, so ≅by (b)_____.• a. ASA; b. CPCTC• a. HL; b. CPCTC• a. SSS; b. CPCTC• a. SAS; b. CPCTC17. Determine which triangles are congruent by AAS using the information in the diagram below.•ΔABF≅ΔEDF•ΔADC≅ΔEBC•ΔABE≅ΔEDA•ΔABE≅ΔCBE18. Complete the proof.Given: bisects ∠EBC and bisects ∠ECC.Prove:ΔEBD≅ΔCBD.•Same-Side Interior Angles Theorem•given•SSS postulate•Triangle Inequality Theorem19. ΔABD≅ΔCBD. Name the theorem or postulate that justifies the congruence.•SAS•AAS•ASA•none of these1. Determine whether each quadrilateral can be a parallelogram. If not, write impossible.a. Two adjacent angles are right angles, but the quadrilateral is not a rectangle.b. All of the angles are congruent.• a. impossible; b. parallelogram• a. parallelogram; b. parallelogram• a. parallelogram; b. impossible• a. impossible; b. impossible2. Which statement is true?•All rectangles are squares.•All quadrilaterals are squares.•All quadrilaterals are parallelograms.•All parallelograms are quadrilaterals.3. Which statement can be used to determine whether quadrilateral XYZW must be a parallelogram?•≅and ≅•≅and ≅•≅and ≅•XW = WZ and XY = YZ4. Choose the best name for the parallelogram and find the measures of the numbered angles.•Square; all numbered angles are equal to 45°.•Rhombus; all numbered angles are equal to 115°.•Rhombus; all numbered angles are equal to 25°.•Square; all numbered angles are equal to 50°.5. Given: quadrilateral ABCD with A(–2, 3), B(2, –4), C(9, 0), D(5, 7). Then ABCD is a rectangle because•the slopes of the sides in pairs are negative reciprocals.•the product of the slopes of the diagonals is –1.•the figure has four vertices.•opposite sides have the same slope.6. Given the parallelogram below, find coordinates for P, without using any new variables.•(a–c, b)•(a + c, b)•(c, b)•(c, a)7. Find the values of the variables for the rectangle. Then find the lengths of the sides.•x = 7, y = 5; side lengths: 70, 45•x = 5, y = 7; side lengths: 33, 94•x = 5, y = 7; side lengths: 50, 63•x = 7, y = 5; side lengths: 45, 458. Determine whether the quadrilateral is a parallelogram. Explain.≅and ≅•Yes; if two opposite sides are congruent, then the quadrilateral is a parallelogram.•No; if the diagonals of a quadrilateral bisect each other, this is not enough to prove that the quadrilateral is a parallelogram.•No; if two opposite sides are congruent, this is not enough to prove that the quadrilateral is a parallelogram.•Yes; if the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.9. Can coordinate geometry be used to prove that opposite sides and in quadrilateral EFGH are congruent?•Yes; use the Distance Formula to show that the diagonals are congruent.•No; you can only show that EF is parallel to GH by using coordinate geometry.•Yes; use the Distance Formula between vertices E and F, and between vertices G and H.•No; you can only find the slopes of EF and GH by using coordinate geometry.10. A square WXYZ has the vertices W(b, b), X(b, –b), Y(–b, –b), and Z(–b, b). Which vertex is in QuadrantII?•W•Z•X•Y11. ∠J and ∠M are base angles of isosceles trapezoid JKLM. If m∠J = 21x + 4, m∠K = 12x– 8, and ∠M = 14x+ 10, find the value of x.•• 2•–•–912. Suppose you are using coordinate geometry to prove that quadrilateral WXYZ is a square. Explain why no twosides should be parallel to the y-axis.•The x-axis would intersect two sides of the square, so the coordinates of the corners would not be clear.•Sides which are parallel to the y-axis would have an undefined slope, so you cannot prove numerically that these sides are parallel.•Points on the sides which are parallel to the y-axis could have any y-value.•The sides of the square which are parallel to the y-axis could be easily confused with the y-axis.13. Find AM if PN = 8 and AO = 5.•8• 3•13• 514. Given square ABCD, where A = (0, a), B = (a, a), C = (0, 0), and D = (a, 0). To prove that the diagonal ADis times the length of side CD, first use _____ to find that = a and = a. Therefore, the ratio= , or .•the definition of isosceles triangle ACD•the definition of right angle C•the Distance Formula•the definition of the origin C = (0, 0)15. A farmer is building a fence for his yard. He is considering two designs, which are shown below. Explain whythe quadrilaterals formed by the horizontal rails and the slanting boards are parallelograms in both designs.•The horizontal rails are parallel to each other. A parallelogram has exactly one pair of parallel sides, so both quadrilaterals are parallelograms.•The horizontal rails are congruent to each other. The slanting boards are all congruent to each other. A parallelogram has two pairs of adjacent sides, but opposite sides are not congruent, so both quadrilaterals are parallelograms.•The horizontal rails are congruent to each other. The slanting boards are all congruent to each other. A parallelogram has four congruent sides, so both quadrilaterals are parallelograms.•The horizontal rails are parallel to each other. The identical slanting boards all slant at the same angle, so the sides are parallel. A parallelogram has both pairs of sides parallel, so both quadrilaterals are parallelograms.16. A rhombus is centered on the origin. One side of the rhombus goes through the points (a, 0) and (0, b). Whatare possible coordinates for one of the other sides?•(–a, 0), (a, 0)•(b, 0), (0, –a)•(0, –b), (0, b)•(–a, 0), (0, –b)17. If a quadrilateral is a parallelogram, then its opposite sides are _____.•perpendicular•adjacent•congruent•none of these18. Find the value of each variable in the parallelogram. m∠1 = 10x, m∠2 = x + y, and m∠3 = 18z.•x = 9, y = 81, z = 5•x = 18, y = 167, z = 5•x = 18, y = 162, z = 10•x = 9, y = 86, z = 019. Complete ≅ _____ for parallelogram EFGH. Then state a definition or theorem as the reason.•; because the angles of a parallelogram bisect each other•; because the diagonals of a parallelogram bisect each other•; because the diagonals of a parallelogram bisect each other•; because the angles of a parallelogram bisect each other20. Which of the following sets of points represents a line segment in Quadrant III and its reflection in the x-axis?(Use the positive numbers a, b, c, d for the coordinates of the endpoints).•(a, b), (c, d); reflection (–a, b), (–c, d)•(–a, –b), (–c, –d); reflection (–a, b), (–c, d)•(a, b), (c, d); reflection (a, –b), (c, –d)•(–a, –b), (–c, –d); reflection (a, –b), (c, –d)1. Solve for a and b.•a = , b =•a = , b =•a = , b =•a = , b =2. State whether ΔADB∼ΔCDB, and if so, identify the theorem that proves the triangles similar.•yes, SSS∼•yes, AA∼•yes, SAS∼•no3. ABCDE∼GHJDF. Complete the congruence and proportion statements.a.∠H≅b.=• a.B; b.AE• a.E; b.DC• a.E; b.AE• a.B; b.DC4. Write a similarity statement for the two triangles.•ΔVUT∼ΔWXY•ΔTVU∼ΔWXY•ΔTUV∼ΔWXY•ΔTUV∼ΔWYX5. Find the geometric mean of 48 and 3.•9•25.5•12•166. The extendable ramp shown below is used to move crates of fruit to loading docks of different heights. When thehorizontal distance AB is 4 feet, the height of the loading dock, BC, is 3 feet. What is the height of the loading dock, DE?•7 ft•9 ft•11 ft7. Find the geometric mean of 20 and 5.•10• 4•12.5•258. In movies and television, the ratio of the width of the screen to the height is called the aspect ratio. Televisionscreens usually have an aspect ratio of 4 : 3, while movie screens usually have an aspect ratio of 1.85 : 1. However, if a movie is made for television in "Letterbox" format, it retains the 1.85 : 1 aspect ratio and fills in the top and bottom parts of the screen with black bars. What would be the height of a movie in "Letterbox" format on a television screen that measures 25 inches along its diagonal? (Hint: First find the width and height of the television screen.)•13.51 in.•10.81 in.•15 in.•8.12 in.9. Use the diagram to determine the height of the tree.•264 ft•72 ft•60 ft•80 ft10. The two rectangles are similar.Which is a correct proportion between corresponding sides?•=•=•=11. Use the Side-Splitter Theorem to find x given that || .•18•12•24• 612. Find OM if bisects ∠NLM, LM = 14, NO = 3, and LN = 4. Round your answer to the nearest hundredth, ifnecessary.•12.27•18.67•0.86•10.513. There is a law that the ratio of the width to length for the American flag should be 10 : 19. Which dimensionsare NOT in the correct ratio?•20 by 38 in.•50 by 95 ft•20 by 44 ft•100 by 190 ft14. If one measurement of a golden rectangle is 6.8 inches, which could be the other measurement?•8.418 in.•11.002 in.• 1.618 in.• 5.182 in.15. If one measurement of a golden rectangle is 8.2 inches, which could be the other measurement?•9.818 in.• 6.582 in.• 1.618 in.• 5.068 in.16. Solve = .•20•19•15•2417. Find OM if bisects ∠NLM, LM =15, NO = 5, and LN = 11. Round your answer to the nearest hundredth, ifnecessary.•33• 6.82• 3.67•8.5918. The width of a golden rectangle is 3 m, which is shorter than the length. What is the length?• 1.85 m• 2.32 m• 3.64 m• 4.85 m19. Find and simplify the ratio of the length to the width of the rectangle.••••20. ΔBGH∼ΔSWQ. What are the pairs of corresponding sides?•BG and SQ, BH and SW, GH and WQ•BG and GB, SQ and QS, GH and HG•BG and SW, BH and SQ, GH and WQ•BG and WQ, BH and SW, GH and SQ1. A building near Atlanta, Georgia, is 181 feet tall. On a particular day at noon it casts a 204-foot shadow. Whatis the sun's angle of elevation at that time?•41.6°•27.5°•62.5°•48.4°2. In right triangle ΔABC, sin A = . What is cos A?••••none of these3. Find the ratio for cos x.••••14. Find the value of x to the nearest meter.•46 m•40 m•35 m•36 m5. Compare the quantity in Column A with the quantity in Column B. The diagram may not be drawn to scale.•The quantity in Column A is greater.•The quantity in Column B is greater.•The two quantities are equal.•The relationship cannot be determined on the basis of the information given.6. How many of these triples could be sides of a right triangle: (27, 36, 45), (12, 17, 20), (24, 32, 40), (14,48, 50)?• 4 triples• 3 triples• 2 triples• 1 triple7. Find the ratio for cos x.•••2•8. Find a third number of the Pythagorean triple that includes 72 and 75.•9•21•37•1049. Find the measure of the marked acute angle to the nearest degree.•62°•28°•61°•118°10. In ΔABC, ∠A is a right angle and m∠B = 60. If AB = 20 ft, find BC. If necessary, round your answer tothe nearest tenth.•10 ft.•40 ft•20 ft•ft11. Find the value of the variable to the nearest hundredth.• 5.28 cm•0.32 cm• 3.13 cm• 5.12 cm12. Find the length of the leg of the right triangle. Leave your answer in simplest radical form.•48••288•13. In ΔABC, ∠A is a right angle and m∠B = 45. If AB = 20 ft, find BC.•10 ft•20 ft•40 ft•20 ft14. Which direction bearing is shown?•19° north of east•19° north of west•19° south of east•19° south of west15. Leslie used the diagram to compute the distance from Ferris to Dunlap to Butte. How much shorter is the distancedirectly from Ferris to Butte than the distance Leslie found?•123 mi•87 mi•36 mi•84 mi16. Which vector has a direction of 31° east of north?••••17. Find the value of x to the nearest tenth.•14.4• 6.3•7.8• 3.118. Find the value of x.•3•6•12• 619. Find the value of x to the nearest tenth.•7.8•18.3•33.0•8.920. Find the value of x to the nearest integer when tan x = 1.483.•58•56•55•571. Which type of isometry is the equivalent of two reflections across two vertical lines?•translation•rotation•glide reflection•none of these2. A section of a tessellated plane is shown below. Which types of symmetry does the tessellated plane have?•glide-reflectional symmetry•translational, rotational, and glide-reflectional symmetry•rotational symmetry•translational and reflectional symmetry3. A blueprint for a house has a scale of 1 : 30. A wall in the blueprint is 7 in. What is the length of the actualwall?•210 ft•21 ft•17.5 ft•none of these4. What is the image of the point (4, –2) after a rotation 270° clockwise about the origin?•(–4, 2)•(4, 2)•(–2, 4)•(2, 4)5. Which graph shows a triangle and its reflection image in the x-axis?••••6. Write a rule to describe a reflection over the y-axis.•(x, y) → (–x, y)•(x, y) → (–x, –y)•(x, y) → (x, –y)•(x, y) → (y, x)•reflectional•rotational•rotational and reflectional•none of these8. Find the image of O(0, 0) after two reflections, first in y = 4, and then in x = –7.•(7, –4)•(–14, 8)•(8, –14)•(–4, 7)9. A section of a tessellated plane is shown below. Which types of symmetry does the tessellated plane have?•rotational symmetry•translational and rotational symmetry•reflectional symmetry•glide-reflectional symmetry•rotational•reflectional•rotational and reflectional•none of these11. Describe the translation 7 units to the left, 12 units up using a vector.•)–12, 7*•)12, –7*•)–7, 12*•)7, –12*12. If the figure has rotational symmetry, find the angle of rotation about the center that results in an imagethat matches the original figure.•120°•90°•72°•It has no rotational symmetry.13. Find the glide reflection image of the solid triangle for the translation )–6, –3* and reflection in y = –1.••••14. If a point P(1, –2) is reflected across the line x = 3, what are the coordinates of its reflection image?•(1, –4)•(1, 8)•(–7, –2)•(5, –2)15. Which translation from thin-lined figure to thick-lined figure is given by the vector )6, 6*?••••16. The dotted triangle is a dilation image of the solid triangle. What is the scale factor?•• 2• 3•17. Find the image of C under the translation described by each vector.a.)4, 5*b.)11, –8*• a.A; b.B• a.B; b.A• a.E; b.D• a.D; b.E18. What is the image of the point (–3, 4) after a rotation of 90° counterclockwise about the point (–3, 0)?•(–7, 0)•(1, 0)•(0, –3)•(–3, –4)19. Which letter has rotational symmetry?• E•X•J•T20. Use scalar multiplication to find the image of the quadrilateral for a dilation with center (0, 0) and scalefactor 2. Graph the quadrilateral and its image.••••。
加拿大数学10年级练习第三部分

加拿大数学10年级练习第三部分1.writey=(x?6)(x?4)(x+2)instandardform.y=x3+48y=x3?8x2+4x+48y=x3?2x2+4x?8y=x3?8x2?8x+242。
因子X4?8x2+15。
(x?3)2(x?5)2(x?3)(x+3)(x?5)(x+5)(x?(x?)(x+)2(x?)(x?)2)(十)+)3.solvex3+5x2?9x?45=0通过绘图。
5,3? 5.3,3? 3,5,3? 5,94.evaluate.60,480504845.找到x3+4x2+3x+12=0的方向。
?x=?4,±ix=?4,x=?4,±3ix=4,±i6.classify2x3?2xbydegreeandbynumberofterms.cubicmonomialquadraticbinomialcubicbinomialcubictrinomial7.使用合成除法进行(5x3?6?5x)÷(10+5x)除法。
x2?2x+3,r24x2?3x,r44x2?2x+3,r?36x2?3x,r?56eagraphingcalculatortofindapolynomialfunctiontomodelthedata.f(x)=4.68x3+8.87x2+4.25x+6.32f(x)=4.68x2+8.87x+6.32f(x)=?4.68x3?8.87x2?4.25倍?6.32f(x)=0.4468x3+8.87x2+4.25x+6.329。
第三因素?813(q?3)(q2+3q+9)(q+3)(q2+3q+9)?第三季度(第二季度+第三季度+9季度) (q3)(q2+3q+9)10.表中显示了自1987年以来杂交棉树木取代树木的数量。
找到一个Cubic函数来对数据进行建模,并使用它来估计1998年种植的棉花数量。
t(x)=0.3x3+0.3x+0.4;167.2Housandtreest(x)=0.3x3+0.3x2+0.1x+0.1;167.2Housandtreest(x)=0.1x3+0.3x2+0.3x+0.1;1728万树(x)=0.1x3+0.1x+0.4;1728万树11。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
GRADE 10 PRINCIPLES OF MATHEMATICS (ACADEMIC)MPM 2DTotal Marks:INSTRUCTIONS:1. Calculators may be used.2. Read all instructions carefully in order to maximize your mark.A/C[K] Part A – Multiple Choice 25 Marks (25 questions * 1 mark each)For each of the following questions in this section, circle the letterrepresenting the correct answer.1. A linear system of two equations that has one solution representstwo lines that are:a) parallel b) coincident c) intersecting d) none of these2. The midpoint of RS is M(8, -1). If point S has coordinates (11, 4) whatare the coordinates of point Ra) (3, -6) b) (15, -6) c) (5, -6) d) (3, 9)3. The midpoint of the line segment with end points A(-8, 8) and B(6, 4) is:a) (0, 10)b) (1, 2)c) (7, 2)d) (-1, 6)4. The equation of a horizontal line passing through the point (4, 2) is:a) 2=xb) 4=yc) 2=yd) 4=x5. The equation of a line with a slope of 5=m and a y intercept of 8 is:a) 85+=x y b) 85+-=x y c) 85--=x y d) 58+=x y6. The slopes of 2 lines are -7 and 71. These lines are said to be:a) parallelb) perpendicular c) coincident d) none of these7.The slope of a line segment passing through 2 points (10,- 4) and (-2, -16) is:a) 1b) 2c) -1d) -28. The length of a line segment with end points (-6, 7) and (-1, -5) is:a) 12b) 5c) 13d) 1699. The diameter of a circle whose equation is 28922=+y x is:a) 15 b) 16c)17d) none of these10.The equation of a circle with a centre of (0, 0) that also passesthrough the point (-8, -6) is:a) 1022=+y x b) 10022=+y xc) 1422=+y xd) 4822=-y x11.The y-intercept of the line 01052=+-y x is:a) 2 b) -2c) 10d) 512.The slope of the line 0124=-+y x is:a) 2 b) -2c) 1d) 013.If (-3, y) is a solution to the equation 132=+y x , what is the valueof ya) 3 b) 6c) 5d) 814.The product ()()z y x z y x 323243-- is equal to:a) 2612z xy b) 26412z y xc)2612z xy - d) 00412z y x15.A simplified expression for ()()n m n m ----52 is:a) m 7 b) n m 27+c) m 3-d) n m 27-16. A simplified expression for 242927abcbc a -- is:a) ac 3 b) abc 3c) 23ac d) 223c a17.The slope of the line, which is perpendicula r to the line, 084=+-y xis:a) -4 b) 4c) 1d) -118.The shortest distance from the point (2, -3) to the line 4-=x is:a) 5 b) 3c) 2d) 619.The value of the polynomial 8542+-a a when 3-=a is:a) 59 b) 44c) 13d) 2920.Which of the following is not a function :a) ()()(){}7,6,5,4,3,2 b) 22x y = c) 22y x =d) ()()(){}3,8,3,7,2,621.The range of the relation whose equation is 52--=x y is:a) 5-≤y b) 5≤yc) 5-≥yd) 5≥y22.The vertex of the parabola ()642--=x y is:a) ()6,4-b) ()6,4-c) ()4,6- d) ()4,6-23. The equation of the axis of symmetry of the parabola ()5242+--=x y is:a) 5=xb) 5-=xc) 2=xd) 2-=x24.A parabola with a vertex of ()3,2 and a stretch factor of 41-(relative to 2x y =) would have an equation of:a) ()32412+--=x y b) ()32412++-=x y c)()23412-+-=x y d) ()23412++-=x y25 The parabola k x y +-=24 passes through the point ()3,2-. T he value ofk is:a) -19 b) 11c) 13d) 19A/CPart B – Short AnswersFor each of the questions in this section, write your answers in the spaces provided . Use the foolscap provided for any rough work. Show details of calculations wherever requested.1. In the accompanying diagram, state each of the following: (4 Marks) [K]a)domain: __________ (1Mark)[K]b) range: __________ (1Mark)[C] c) Is the relation a function Justify your answer. (2 marks)[A]2. The x-intercepts of the parabola 2892-=x y are: __________ and __________.(Show your work) (2 Marks)[A]3. The roots of the quadratic equation 0101732=+-x x are: __________ and __________.(Show your work) (3 Marks)[A]4. Write the equation of the parabola with a vertex of (4, 23) if it passes through the point (-1, -2): (Show your work) (3 Marks)____________________[T] 5. A line passes through 2 points (1, 4) and (2,-4). Calculate the slope of the line . Also show the equation of the line in the form 0=++C By Ax . (Show your work) (4 Marks)____________________ ____________________SlopeEquation[K] 6. The Tangent of 45 is: __________ (1 Mark)[A]7. a) In the accompanying diagram, the two triangles are similar . What is the value of x(Show your work) (2 Marks)=x __________[T]b) If the area of the smaller triangle is 8 cm 2, what is the area of the larger triangle(Show your work) ( 2 Marks)Area = __________[K]8 Given that sin A = 21, find A ∠ (to the nearest degree) __________(1 Mark)[A] 9. In the accompanying right triangle , find the value of x to one decimal place.(Show your work) (2 Marks)=x ________[A] 10. Use the SINE LAW to find the value of side x to one decimal place. (Show your work) (2 Marks)x = ________3028x5642x30[A] 11. Use the COSINE LAW to find the value of side x to one decimalplace.(Show your work) (2 Marks)x = ________[T]12.Factor each of the following to the fullest extent possible: (4Questions * 2 marks each)a) y x my mx 22--+________________________b) 31142--x x________________________c) 2416916y x -________________________d) 2225309s rs r +-________________________A/CPart C – Full Solutions Required562030 xFor each of the questions in this section, full solutions are required. Record your answers in the spaces provided. Use the foolscap provided for any rough work.[A]1. Solve the linear system using the elimination method . Remember to find values for both x and y. (5 Marks)225=+y x2132-=-y x[C]Explain what the solution above represents geometrically. How do you know that the solution you arrived at is the correct answer (2 Marks)[A] 2. Expand and simplify the polynomial ()()()21432+-+-x x x . (4 Marks)[T]3. Find the equation of the line perpendicular to the line 088=-+y x and passing through the point (-4, 1). (4 Marks)[T] 4. From the window of one building, a man finds that the angle of elevation to the top of a second building is 47 and the angle ofdepression to the bottom of the same building is 58. The buildingsare 60 m apart. Find the height of the 2nd building to the nearestmetre. A diagram is required. (6 Marks)[T] 5. ABC has vertices A(1, 7), B(-5, 3) and C(3, -1). Determine the equation for AE, the altitude from vertex A to the opposite side BC.(5 Marks)6. The hypotenuse of a right triangle is 26 cm. The sum of the othertwo sides is 34 cm. (9 Marks)[T] a) Find the length of the other two sides of the triangle. (3 Marks)[T] b) Find the measure of the other two angles. Round to the nearest degree. (3 Marks)[C] c) Describe a situation where you would be able to use knowledge ofthe Pythagorean theorem in a practical, real life situation. (3Marks)[T] 7. A rectangular skating rink measures 20m by 20m. It has been decidedto increase the area of the rink by a factor of 4. Determine howmuch each side should be extended. Assume that each side isextended by the same amount. (6 Marks)[C] What is the significance of keeping the skating rink in the shape ofa square Justify your answer. (3 Marks)[A] 8. a) Solve 35122+=d d using the quadratic formula. (2 Marks)[A] b) Solve 0x by factoring. Check your solutions. (2 Marks)-122=3。
