加拿大安大略省十年级数学试卷-英文
加拿大国际袋鼠数学竞赛试题及答案-2016年ParentsQuestions

加拿⼤国际袋⿏数学竞赛试题及答案-2016年ParentsQuestionsCanadian Math Kangaroo ContestPart A: Each correct answer is worth 3 points1.Which letter on the board is not in the word "KOALA"?(A) R (B) L (C) K (D) N (E) O2.In a cave, there were only two seahorses, one starfish and three turtles. Later, five seahorses, three starfishand four turtles joined them. How many sea animals gathered in the cave?(A) 6 (B) 9 (C) 12 (D) 15 (E) 183.Matt had to deliver flyers about recycling to all houses numbered from 25 to 57. How many houses got theflyers?(A) 31 (B) 32 (C) 33 (D) 34 (E) 354.Kanga is 1 year and 3 months old now. In how many months will Kanga be 2 years old?(A) 3 (B) 5 (C) 7 (D) 8 (E) 95.(A) 24 (B) 28 (C) 36 (D) 56 (E) 806. A thread of length 10 cm is folded into equal parts as shown in the figure.The thread is cut at the two marked places. What are the lengths of the three parts?(A) 2 cm, 3 cm, 5 cm (B) 2 cm, 2 cm, 6 cm (C) 1 cm, 4 cm, 5 cm(D) 1 cm, 3 cm, 6 cm (E) 3 cm, 3 cm, 4 cm7.Which of the following traffic signs has the largest number of lines of symmetry?(A) (B) (C) (D) (E)8.Kanga combines 555 groups of 9 stones into a single pile. She then splits the resulting pile into groups of 5 stones. How many groups does she get?(A) 999 (B) 900 (C) 555 (D) 111 (E) 459.What is the shaded area?(A) 50 (B) 80 (C) 100 (D) 120 (E) 15010.In a coordinate system four of the following points are the vertices of a square. Which point is not a vertexof this square?(A) (?1;3)(B) (0;?4)(C) (?2;?1)(D) (1;1)(E) (3;?2)Part B: Each correct answer is worth 4 points11.There are twelve rooms in a building and each room has two windows and one light. Last evening, eighteen windows were lighted. In how many rooms was the light off?(A) 2 (B) 3 (C) 4 (D) 5 (E) 612.Which three of the five jigsaw pieces shown can be joined together to form a square?(A) 1, 3 and 5 (B) 1, 2 and 5 (C) 1, 4 and 5 (D) 3, 4 and 5 (E) 2, 3 and 513.John has a board with 11 squares. He puts a coin in each of eight neighbouring squareswithout leaving any empty squares between the coins. What is the maximum numberof squares in which one can be sure that there is a coin?(A) 1 (B) 3 (C) 4 (D) 5 (E) 614.Which of the following figures cannot be formed by gluing these two identical squares of paper together?(A) (B) (C) (D) (E)15.Each letter in BENJAMIN represents one of the digits 1, 2, 3, 4, 5, 6 or 7. Different letters represent different digits. The number BENJAMIN is odd and divisible by 3. Which digit corresponds to N?(A) 1 (B) 2 (C) 3 (D) 5 (E) 716.Seven standard dice are glued together to make the solid shown. The faces of the dice thatare glued together have the same number of dots on them. How many dots are on the surfaceof the solid?(A) 24 (B) 90 (C) 95 (D) 105 (E) 12617.Jill is making a magic multiplication square using the numbers 1, 2, 4, 5, 10, 20, 25, 50 and 100. The productsof the numbers in each row, in each column and in the two diagonals should all be the same. In the figure you can see how she has started. Which number should Jill place in the cell with the question mark?(A) 2 (B) 4 (C) 5 (D) 10 (E) 2518.What is the smallest number of planes that are needed to enclose a bounded part in three-dimensional space?(A) 3 (B) 4 (C) 5 (D) 6 (E) 719.Each of ten points in the figure is marked with either 0 or 1 or 2. It is known thatthe sum of numbers in the vertices of any white triangle is divisible by 3, while thesum of numbers in the vertices of any black triangle is not divisible by 3. Three ofthe points are marked as shown in the figure. What numbers can be used to markthe central point?(A) Only 0. (B) Only 1. (C) Only 2. (D) Only 0 and 1. (E) Either 0 or 1 or 2.20.Betina draws five points AA,BB,CC,DD and EE on a circle as well as the tangent tothe circle at AA, such that all five angles marked with xx are equal. (Note thatthe drawing is not to scale.) How large is the angle ∠AABBDD ?(A) 66°(B) 70.5°(C) 72°(D) 75°(E) 77.5°Part C: Each correct answer is worth 5 points21.Which pattern can we make using all five cards given below?(A) (B) (C) (D) (E)22.The numbers 1, 5, 8, 9, 10, 12 and 15 are distributed into groups with one or more numbers. The sum of thenumbers in each group is the same. What is the largest number of groups?(A) 2 (B) 3 (C) 4 (D) 5 (E) 623.My dogs have 18 more legs than noses. How many dogs do I have?(A) 4 (B) 5 (C) 6 (D) 8 (E) 924.In the picture you see 5 ladybirds.Each one sits on its flower. Their places are defined as follows: the difference of the dots on their wings is the number of the leaves and the sum of the dots on their wings is the number of the petals. Which of the following flowers has no ladybird?(A) (B) (C) (D) (E)25.On each of six faces of a cube there is one of the following six symbols: ?, ?, ?, ?, ? and Ο. On each face there is a different symbol. In the picture we can see this cube shown in two different positions.Which symbol is opposite the ??(A) Ο(B)?(C) ?(D) ?(E) ?26.What is the greatest number of shapes of the form that can be cut out from a5 × 5 square?(A) 2 (B) 4 (C) 5 (D) 6 (E) 727.Kirsten wrote numbers in 5 of the 10 circles as shown in the figure. She wants to writea number in each of the remaining 5 circles such that the sums of the 3 numbers alongeach side of the pentagon are equal. Which number will she have to write in the circlemarked by XX?(A) 7 (B) 8 (C) 11 (D) 13 (E) 1528. A 3×3×3 cube is built from 15 black cubes and 12 white cubes. Five faces of the larger cube are shown.Which of the following is the sixth face of the large cube?(A) (B) (C) (D) (E)29.Jakob wrote down four consecutive positive integers. He then calculated the four possible totals made bytaking three of the integers at a time. None of these totals was a prime. What is the smallest integer Jakob could have written?(A) 12 (B) 10 (C) 7 (D) 6 (E) 330.Four sportsmen and sportswomen - a skier, a speed skater, a hockey player and a snowboarder - had dinnerat a round table. The skier sat at Andrea's left hand. The speed skater sat opposite Ben. Eva and Filip sat next to each other.A woman sat at the hockey player`s left hand. Which sport did Eva do?(A) speed skating (B) skiing (C) ice hockey (D) snowboarding(E) It`s not possible to find out with the given information.International Contest-Game Math Kangaroo Canada, 2016Answer KeyParents Contest。
AMC 10A 2017 英语题目及答案

2017 AMC 10A ProblemsContents[hide]▪1 Problem 1▪2 Problem 2▪3 Problem 3▪4 Problem 4▪5 Problem 5▪6 Problem 6▪7 Problem 7▪8 Problem 8▪9 Problem 9▪10 Problem 10▪11 Problem 11▪12 Problem 12▪13 Problem 13▪14 Problem 14▪15 Problem 15▪16 Problem 16▪17 Problem 17▪18 Problem 18▪19 Problem 19▪20 Problem 20▪21 Problem 21▪22 Problem 22▪23 Problem 23▪24 Problem 24▪25 Problem 25▪26 See alsoProblem 1What is the value of ?SolutionProblem 2Pablo buys popsicles for his friends. The store sells single popsicles for each, -popsicle boxes for each, and -popsicle boxes for . What is the greatest number of popsicles that Pablo can buy with ?SolutionProblem 3Tamara has three rows of two -feet by -feet flower beds in her garden. The beds are separated and also surrounded by -foot-wide walkways, as shown on the diagram. What is the total area of the walkways, in square feet?Problem 4Mia is “helping” her mom pick up toys that are strewn on the floor. Mia’s mom manages to put toys into the toy box every seconds, but each time immediately after those seconds have elapsed, Mia takes toys out of the box. How much time, in minutes, will it take Mia and her mom to put all toysinto the box for the first time?Problem 5The sum of two nonzero real numbers is times their product. What is the sum ofthe reciprocals of the two numbers?SolutionMs. Carroll promised that anyone who got all the multiple choice questions right on the upcoming exam would receive an A on the exam. Which of of these statements necessarily follows logically?SolutionProblem 7Jerry and Silvia wanted to go from the southwest corner of a square field to the northeast corner. Jerry walked due east and then due north to reach the goal, but Silvia headed northeast and reached the goal walking in a straight line. Which of the following is closest to how much shorter Silvia's trip was, compared to Jerry's trip?SolutionProblem 8At a gathering of people, there are people who all know each other and people who know no one. People who know each other hug, and people who do not know each other shake hands. How many handshakes occur?SolutionProblem 9Minnie rides on a flat road at kilometers per hour (kph), downhill at kph, and uphill at kph. Penny rides on a flat road at kph, downhill at kph, and uphill at kph. Minnie goes from town to town , a distance of km all uphill, then from town to town , a distance of km all downhill, and then back to town , a distance of km on the flat. Penny goes the other way around using the same route. How many more minutes does it take Minnie to complete the -km ride than it takes Penny?SolutionJoy has thin rods, one each of every integer length from cm through cm. She places the rods with lengths cm, cm, and cm on a table. She then wants to choose a fourth rod that she can put with these three to form a quadrilateral with positive area. How many of the remaining rods can she choose as the fourth rod?SolutionProblem 11The region consisting of all points in three-dimensional space within units of line segment has volume . What is the length ?SolutionProblem 12Let be a set of points in the coordinate plane such that two of the three quantities and are equal and the third of the three quantities is no greater than this common value. Which of the following is a correct description forSolutionProblem 13Define a sequence recursively by and the remainder when is divided by for all Thus the sequence startsWhat isSolutionProblem 14Every week Roger pays for a movie ticket and a soda out of his allowance. Last week, Roger's allowance was dollars. The cost of his movie ticket was of the difference between and the cost of his soda, while the cost of his soda was of the difference between and the cost of his movie ticket. To the nearest whole percent, what fraction of did Roger pay for his movie ticket and soda?SolutionProblem 15Chloé chooses a real number uniformly at random from the interval . Independently, Laurent chooses a real number uniformly at random from the interval . What is the probability that Laurent's number is greater than Chloé's number?SolutionProblem 16There are 10 horses, named Horse 1, Horse 2, , Horse 10. They get their names from how many minutes it takes them to run one lap around a circular race track: Horse runs one lap in exactly minutes. At time 0 all the horses are together at the starting point on the track. The horses start running in the same direction, and they keep running around the circular track at their constant speeds. The least time , in minutes, at which all 10 horses will again simultaneously be at the starting point is . Let be the least time, in minutes, such that at least 5 of the horses are again at the starting point. What is the sum of the digits of ?SolutionProblem 17Distinct points , , , lie on the circle and have integer coordinates. The distances and are irrational numbers. What is the greatest possible value of the ratio ?SolutionProblem 18Amelia has a coin that lands heads with probability , and Blaine has a coin thatlands on heads with probability . Amelia and Blaine alternately toss their coins until someone gets a head; the first one to get a head wins. All coin tosses are independent. Amelia goes first. The probability that Amelia wins is , where and are relatively prime positive integers. What is ?SolutionProblem 19Alice refuses to sit next to either Bob or Carla. Derek refuses to sit next to Eric. How many ways are there for the five of them to sit in a row of 5 chairs under these conditions?SolutionProblem 20Let equal the sum of the digits of positive integer . For example,. For a particular positive integer , . Which of the following could be the value of ?SolutionProblem 21A square with side length is inscribed in a right triangle with sides of length , , and so that one vertex of the square coincides with the right-angle vertex of the triangle. A square with side length is inscribed in another right triangle with sides of length , , and so that one side of the square lies on the hypotenuseof the triangle. What is ?SolutionProblem 22Sides and of equilateral triangle are tangent to a circle at points and respectively. What fraction of the area of lies outside the circle?SolutionProblem 23How many triangles with positive area have all their vertices at points in the coordinate plane, where and are integers between and , inclusive?SolutionProblem 24For certain real numbers , , and , the polynomialhas three distinct roots, and each root of is also a root of the polynomial What isSolutionProblem 25How many integers between and , inclusive, have the property that some permutation of its digits is a multiple of between and For example, both and have this property.Solution2017 AMC 10A Answer Key1. C2. D3. B4. B5. C6. B7. A8. B9. C10. B11. D12. E13. D14. D15. C16. B17. D18. D19. C20. D21. D22. E23. B24. C25. A2017 AMC 10A Problems/Problem 1Contents[hide]▪1 Problem▪2 Solution 1▪3 Solution 2▪4 Solution 3▪5 Solution 4▪6 See AlsoProblemWhat is the value of ?Solution 1Notice this is the term in a recursive sequence, defined recursively asThus:Solution 2Starting to compute the inner expressions, we see the results are . This is always less than a power of . The only admissible answer choice by this rule is thus .Solution 3Working our way from the innermost parenthesis outwards and directly computing, we have .Solution 4If you distribute this you get a sum of the powers of . The largest power of in the series is , so the sum is .2017 AMC 10A Problems/Problem 2 ProblemPablo buys popsicles for his friends. The store sells single popsicles for each, -popsicle boxes for each, and -popsicle boxes for . What is the greatest number of popsicles that Pablo can buy with ?Solutionboxes give us the most popsicles/dollar, so we want to buy as many of those as possible. After buying , we have left. We cannot buy a third box, so we opt for the box instead (since it has a higher popsicles/dollar ratio than the pack). We're now out of money. We bought popsicles, so theanswer is .2017 AMC 10A Problems/Problem 3 ProblemTamara has three rows of two -feet by -feet flower beds in her garden. The beds are separated and also surrounded by -foot-wide walkways, as shown on the diagram. What is the total area of the walkways, in square feet?Finding the area of the shaded walkway can be achieved by computing the total area of Tamara's garden and then subtracting the combined area of her six flower beds.Since the width of Tamara's garden contains three margins, the total width isfeet.Similarly, the height of Tamara's garden is feet.Therefore, the total area of the garden is square feet.Finally, since the six flower beds each have an area of square feet, the area we seek is , and our answer is2017 AMC 10A Problems/Problem 4Contents[hide]▪1 Problem▪2 Solution▪3 Solution 2▪4 See alsoProblemMia is helping her mom pick up toys that are strewn on the floor. Mia’s mom manages to put toys into the toy box every seconds, but each time immediately after those seconds have elapsed, Mia takes toys out of the box. How much time, in minutes, will it take Mia and her mom to put all toys into the box for the first time?SolutionEvery seconds toys are put in the box, so after seconds there will be toys in the box. Mia's mom will then put toys into to the box and we have our total amount of time to be seconds, whichequals minutes.Solution 2Though Mia's mom places toys every seconds, Mia takes out toys right after. Therefore, after seconds, the two have collectively placed toy into the box. Therefore by minutes, the two would have placed toys into the box. Therefore, at minutes, the two would have placed toys into the box. Though Mia may take toys out right after, the number of toys in the box firstreaches by minutes.2017 AMC 10A Problems/Problem 5Contents[hide]▪1 Problem▪2 Solution▪3 Solution 2▪4 Solution 3▪5 See AlsoProblemThe sum of two nonzero real numbers is 4 times their product. What is the sum of the reciprocals of the two numbers?SolutionLet the two real numbers be . We are given that and dividing both sides by ,Note: we can easily verify that this is the correct answer; for example, 1/2 and 1/2 work, and the sum of their reciprocals is 4.Solution 2Instead of using algebra, another approach at this problem would be to notice the fact that one of the nonzero numbers has to be a fraction. See for yourself. Andby looking into fractions, we immediately see that and would fit the rule.Solution 3Notice that from the information given above,Because the sum of the reciprocals of two numbers is just the sum of the two numbers over the product of the two numbers orWe can solve this by substituting .Our answer is simply .Therefore, the answer is .2017 AMC 10A Problems/Problem 6 ProblemMs. Carroll promised that anyone who got all the multiple choice questions right on the upcoming exam would receive an A on the exam. Which one of these statements necessarily follows logically?SolutionRewriting the given statement: "if someone got all the multiple choice questions right on the upcoming exam then he or she would receive an A on the exam." If that someone is Lewis the statement becomes: "if Lewis got all the multiple choice questions right, then he got an A on the exam." The contrapositive: "If Lewis did not receive an A, then he got at least one of the multiple choice questions wrong (did not get all of them right)" must also be true leaving B as the correct answer. B is also equivalent to the contrapositive of the original statement, which implies that it must be true, so the answer is.2017 AMC 10A Problems/Problem 7 ProblemJerry and Silvia wanted to go from the southwest corner of a square field to the northeast corner. Jerry walked due east and then due north to reach the goal, but Silvia headed northeast and reached the goal walking in a straight line. Which ofthe following is closest to how much shorter Silvia's trip was, compared to Jerry's trip?SolutionLet represent how far Jerry walked, and represent how far Sylvia walked. Since the field is a square, and Jerry walked two sides of it, while Silvia walked the diagonal, we can simply define the side of the square field to be one, and find the distances they walked. Since Jerry walked two sides, Since Silvia walked the diagonal, she walked the hypotenuse of a 45, 45, 90 triangle with leglength 1. Thus, We can then take2017 AMC 10A Problems/Problem 8 Contents[hide]▪1 Problem▪2 Solution 1▪3 Solution 2▪4 Solution 3▪5 See AlsoProblemAt a gathering of people, there are people who all know each other and people who know no one. People who know each other a hug, and people who do not know each other shake hands. How many handshakes occur?Solution 1Each one of the ten people has to shake hands with all the other people they don’t know. So . From there, we calculate how many handshakes occurred between the people who don’t know each other. This is simply countinghow many ways to choose two people to shake hands, or . Thus the answer is .Solution 2We can also use complementary counting. First of all, handshakes or hugs occur. Then, if we can find the number of hugs, then we can subtract it from to find the handshakes. Hugs only happen between the 20 people whoknow each other, so there are hugs. . Solution 3We can focus on how many handshakes the 10 people get.The 1st person gets 29 handshakes.2nd gets 28......And the 10th receives 20 handshakes.We can write this as the sum of an arithmetic sequence.Therefore, the answer is2017 AMC 10A Problems/Problem 9 ProblemMinnie rides on a flat road at kilometers per hour (kph), downhill at kph, and uphill at kph. Penny rides on a flat road at kph, downhill at kph, and uphill at kph. Minnie goes from town to town , a distance of km all uphill, then from town to town , a distance of km all downhill, and then back to town , a distance of km on the flat. Penny goes the other way around using the same route. How many more minutes does it take Minnie to complete the -km ride than it takes Penny?SolutionThe distance from town to town is km uphill, and since Minnie rides uphill at a speed of kph, it will take her hours. Next, she will ride from town to town , a distance of km all downhill. Since Minnie rides downhill at a speed of kph, it will take her half an hour. Finally, she rides from town back to town , a flat distance of km. Minnie rides on a flat road at kph, so this will take her hour. Her entire trip takes her hours. Secondly, Penny will go from town to town , a flat distance of km. Since Penny rides on a flat road at kph,it will take her of an hour. Next Penny will go from town to town , which is uphill for Penny. Since Penny rides at a speed of kph uphill, and town and are km apart, it will take her hours. Finally, Penny goes from Townback to town , a distance of km downhill. Since Penny rides downhill atkph, it will only take her of an hour. In total, it takes her hours, which simplifies to hours and minutes. Finally, Penny's Hour Minute trip was minutes less than Minnie's Hour Minute Trip2017 AMC 10A Problems/Problem 10 ProblemJoy has thin rods, one each of every integer length from cm through cm. She places the rods with lengths cm, cm, and cm on a table. She then wants to choose a fourth rod that she can put with these three to form a quadrilateral with positive area. How many of the remaining rods can she choose as the fourth rod?SolutionThe triangle inequality generalizes to all polygons, so andto get . Now, we know that there are numbers between and exclusive, but we must subtract to account for the 2 lengthsalready used that are between those numbers, which gives2017 AMC 10A Problems/Problem 11Contents[hide]▪1 Problem▪2 Solution▪3 Diagram▪4 See AlsoProblemThe region consisting of all point in three-dimensional space within 3 units of line segment has volume 216. What is the length ?SolutionIn order to solve this problem, we must first visualize what the region contained looks like. We know that, in a three dimensional plane, the region consisting of all points within units of a point would be a sphere with radius . However, we need to find the region containing all points within 3 units of a segment. It can be seen that our region is a cylinder with two hemispheres on either end. We know the volume of our region, so we set up the following equation (the volume of our cylinder + the volume of our two hemispheres will equal ):, where is equal to the length of our line segment. Solving, we find that .Diagram/cwNt293.png2017 AMC 10A Problems/Problem 12ProblemLet be a set of points in the coordinate plane such that two of the three quantities and are equal and the third of the three quantities is no greater than this common value. Which of the following is a correct description forSolutionIf the two equal values are and , then . Also, because 3 is the common value. Solving for , we get . Therefore the portion of the line where is part of . This is a ray with an endpoint of .Similar to the process above, we assume that the two equal values are and . Solving the equation then . Also, because 3 is the common value. Solving for , we get . Therefore the portion of the line where is also part of . This is another ray with the sameendpoint as the above ray: .If and are the two equal values, then . Solving the equation for , we get . Also because is one way toexpress the common value. Solving for , we get . Therefore the portion of the line where is part of like the other two rays. Thelowest possible value that can be achieved is also .Since is made up of three rays with common endpoint , the answer is2017 AMC 10A Problems/Problem 13 ProblemDefine a sequence recursively by and the remainder when is divided by for all Thus the sequence startsWhat isSolutionA pattern starts to emerge as the function is continued. The repeating pattern isThe problem asks for the sum of eight consecutive terms in the sequence. Because there are eight numbers in the repeating sequence, we just need to find the sum of the numbers in the sequence, which is2017 AMC 10A Problems/Problem 14 ProblemEvery week Roger pays for a movie ticket and a soda out of his allowance. Last week, Roger's allowance was dollars. The cost of his movie ticket was of the difference between and the cost of his soda, while the cost of his soda was of the difference between and the cost of his movie ticket. To the nearest whole percent, what fraction of did Roger pay for his movie ticket and soda?SolutionLet = cost of movie ticketLet = cost of sodaWe can create two equations:Substituting we get:which yields:Now we can find s and we get:Since we want to find what fraction of did Roger pay for his movie ticket and soda, we add and to get:2017 AMC 10A Problems/Problem 15 Contents[hide]▪ 1 Problem▪ 2 Solution 1▪ 3 Solution 2▪ 4 Solution 3:▪ 5 See AlsoProblemChloé chooses a real number uniformly at random from the interval . Independently, Laurent chooses a real number uniformly at random from the interval . What is the probability that Laurent's number is greater than Chloé's number?Solution 1Denote "winning" to mean "picking a greater number". There is a chance that Laurent chooses a number in the interval . In this case, Chloécannot possibly win, since the maximum number she can pick is . Otherwise, if Laurent picks a number in the interval , with probability , then the two people are symmetric, and each has a chance of winning. Then,the total probability isSolution 2We can use geometric probability to solve this. Suppose a point lies in the-plane. Let be Chloe's number and be Laurent's number. Then obviously we want , which basically gives us a region above a line. We know thatChloe's number is in the interval and Laurent's number is in the interval , so we can create a rectangle in the plane, whose length is andwhose width is . Drawing it out, we see that it is easier to find the probability that Chloe's number is greater than Laurent's number and subtract this probability from . The probability that Chloe's number is larger than Laurent's number issimply the area of the region under the line , which is . Instead of bashing this out we know that the rectangle has area . Sothe probability that Laurent has a smaller number is . Simplifying the expression yields and so .Solution 3:Scale down by to get that Chloe picks from and Laurent picks from . There are an infinite number of cases for the number that Chloe picks, butthey are all centered around the average of . Therefore, Laurent has a range of to to pick from, on average, which is a length of out of a total length of . Therefore, the probability is2017 AMC 10A Problems/Problem 16 Contents[hide]▪ 1 Problem▪ 2 Solution 1▪ 3 Solution 2▪ 4 See AlsoProblemThere are 10 horses, named Horse , Horse , . . . , Horse . They get their names from how many minutes it takes them to run one lap around a circular race track: Horse runs one lap in exactly minutes. At time all the horses are together at the starting point on the track. The horses start running in the same direction, and they keep running around the circular track at their constant speeds. The least time , in minutes, at which all horses will again simultaneously be at the starting point is . Let be the least time, in minutes, such that at least 5 of the horses are again at the starting point. What is the sum of the digits ofSolution 1If we have horses, , then any number that is a multiple of the all those numbers is a time when all horses will meet at the starting point. The least of these numbers is the LCM. To minimize the LCM, we need the smallest primes, and we need to repeat them a lot. By inspection, we find that. Finally, .Solution 2We are trying to find the smallest number that has one-digit divisors. Therefore we try to find the LCM for smaller digits, such as ,, , or . We quickly consider since it is the smallest number that is the LCM of , , and . Since has single-digit divisors, namely , , , , and , our answer is2017 AMC 10A Problems/Problem 17 ProblemDistinct points , , , lie on the circle and have integer coordinates. The distances and are irrational numbers. What is thegreatest possible value of the ratio ?SolutionBecause , , , and are integers there are only a few coordinates that actually satisfy the equation. The coordinates areand We want to maximize and minimize They also have to bethe square root of something, because they are both irrational. The greatest value of happens when it and are almost directly across from each other and are in different quadrants. For example, the endpoints of the segmentcould be and because the two points are almost across from each other. The least value of is when the two endpoints are in the samequadrant and are very close to each other. This can occur when, for example,is and is They are in the same quadrant and no other point on the circle with integer coordinates is closer to the point than Using the distance formula, we get that is and that is2017 AMC 10A Problems/Problem 18 Contents[hide]▪ 1 Problem▪ 2 Solution▪ 3 Solution 2▪ 4 See AlsoProblemAmelia has a coin that lands heads with probability , and Blaine has a coin that lands on heads with probability . Amelia and Blaine alternately toss their coins until someone gets a head; the first one to get a head wins. All coin tosses are independent. Amelia goes first. The probability that Amelia wins is , whereand are relatively prime positive integers. What is ?SolutionLet be the probability Amelia wins. Note that, as if she gets to her turn again, she is back where she started with probability of winning . The chance she wins on her first turn is . The chance she makes itto her turn again is a combination of her failing to win the first turn - and Blaine failing to win - . Multiplying gives us . Thus, Therefore, , so the answer is .Solution 2Let be the probability Amelia wins. Note thatThis can be represented by an infinite geometric series,. Therefore, , so the answer isSolution by ktong2017 AMC 10A Problems/Problem 19 Contents[hide]▪ 1 Problem▪ 2 Solution 1▪ 3 Solution 2▪ 4 See AlsoProblemAlice refuses to sit next to either Bob or Carla. Derek refuses to sit next to Eric. How many ways are there for the five of them to sit in a row of 5 chairs under these conditions?Solution 1For notation purposes, let Alice be A, Bob be B, Carla be C, Derek be D, and Eric be E. We can split this problem up into two cases:A sits on an edge seat.Then, since B and C can't sit next to A, that must mean either D or E sits next to A. After we pick either D or E, then either B or C must sit next to D/E. Then, we can arrange the two remaining people in two ways. Since there are two different edge seats that A can sit in, there are a total of .A does not sit in an edge seat.In this case, then only two people that can sit next to A are D and E, and there are two ways to permute them, and this also handles the restriction that D can't sit next to E. Then, there are two ways to arrange B and C, the remaining people. However, there are three initial seats that A can sit in, so there areseatings in this case.Adding up all the cases, we have .Solution 2Label the seats through . The number of ways to seat Derek and Eric in the five seats with no restrictions is . The number of ways to seat Derek and Eric such that they sit next to each other is (which can be figure out quickly), so the number of ways such that Derek and Eric don't sit next to each other is . Note that once Derek and Eric are seated, there are three cases.The first case is that they sit at each end. There are two ways to seat Derek and Eric. But this is impossible because then Alice, Bob, and Carla would have to sit in some order in the middle three seats which would lead to Alice sitting next to Bob or Carla, a contradiction. So this case gives us ways.Another possible case is if Derek and Eric seat in seats and in some order. There are 2 possible ways to seat Derek and Eric like this. This leaves Alice, Bob, and Carla to sit in any order in the remaining three seats. Since no two of these three seats are consecutive, there are ways to do this. So the second case gives us total ways for the second case.The last case is if once Derek and Eric are seated, exactly one pair of consecutive seats is available. There are ways to seat Derek and Eric like this. Once they are seated like this, Alice cannot not sit in one of the two consecutive available seats without sitting next to Bob and Carla. So Alice has to sit in the other remaining chair. Then, there are two ways to seat Bob and Carla in the remaining two seats (which are consecutive). So this case gives us ways.So in total there are . So our answer is .。
国际学校数学十年级试卷

1. The prime factorisation form of 156
A) 2 ´ 3´ 13
B) 22 ´ 32 ´ 13
2. The logarithemic form of 8x = 2
Chapter Real numbers
2.
Sets
3.
Polynomials
Weightage % 48% 20% 32% 100%
Marks 12 5 8 25
Table : 3 Weightage to difficulty level
S. No.
Difficulty level
Weightage % Marks
A) logx 8 = 2
B) log 2 = x 8
p 3. The q form of the decimal 0.875
7
A) 23
7
B) 16
C) 22 ´ 3 ´ 13 C) log 2 = 8
x
3
C) 8
() D) 2 ´ 32 ´ 13
() D) log x = 8
2
7
D) 22
()
3
1.
Easy
24%
06
2.
Average
3.
Difficulty
52%
13
24%
06
100%
25
Table : 4 Weightage to type of questions
S. No. Type of Questions
Weightage % No. of Questions Marks
amc10英语真题及答案新课标

amc10英语真题及答案新课标AMC10是美国数学竞赛(American Mathematics Competitions)的10年级级别,面向10年级及以下的学生。
以下是一套模拟的AMC10英语真题及答案,请注意,这只是一个示例,并非真实的AMC10题目。
AMC10 英语真题及答案Part 1: Multiple Choice1. The word "innovate" is most closely related to which of the following?A. InnovatorB. InventionC. InventionistD. InnovatoryAnswer: D. Innovatory2. Which of the following is the correct spelling of the word meaning "to make something new"?A. InnovateB. InnovateC. InnovateD. InnovateAnswer: A. Innovate3. The phrase "a leap of faith" is used to describe:A. A large jumpB. A risky decisionC. A new religionD. A sudden increaseAnswer: B. A risky decision4. In the sentence "The company is looking to streamline its operations," the word "streamline" means:A. To make more expensiveB. To make more efficientC. To make more complicatedD. To make more visibleAnswer: B. To make more efficient5. The word "altruistic" is an antonym for:A. SelfishB. AltruismC. AltruisticallyD. AltruistAnswer: A. SelfishPart 2: Fill in the Blanks6. The scientist was awarded the Nobel Prize for his _______ contributions to the field of physics.Answer: innovative7. The _______ of the old building was a significantachievement for the preservation society.Answer: renovation8. The _______ of the new policy was met with mixed reactions from the public.Answer: implementation9. The _______ of the company's profits was due to a series of successful marketing campaigns.Answer: increase10. The _______ of the ancient ruins provided valuable insights into the history of the civilization.Answer: excavationPart 3: Reading ComprehensionRead the following passage and answer the questions that follow.Passage:In recent years, there has been a significant increase in the number of people who are interested in sustainable living. This trend has led to the development of various eco-friendly products and practices. One such practice is the use of solar panels to generate electricity. Solar panels are becoming more popular due to their ability to harness the power of the sun and convert it into usable energy.Questions:11. What is the main topic of the passage?Answer: Sustainable living and the use of solar panels.12. Why are solar panels becoming more popular?Answer: Because they can harness the power of the sun and convert it into usable energy.13. What is the trend mentioned in the passage?Answer: An increase in the number of people interested in sustainable living.Part 4: Vocabulary in Context14. The _______ of the old factory was a major concern for the environmentalists.Answer: pollution15. The _______ of the new technology was celebrated by the scientific community.Answer: advancement16. The _______ of the endangered species was a top priority for the conservation organization.Answer: preservation17. The _______ of the ancient artifact provided evidence ofa previously unknown civilization.Answer: discovery18. The _______ of the new policy was met with skepticism by some members of the community.Answer: enforcement请注意,AMC10是一个数学竞赛,通常不包含英语题目。
加拿大国际袋鼠数学竞赛试题-2013年

(A) 12
(B) 8
(C) 4
(D) 2
(E) 0
3. Which of the dresses has less than seven dots, but more than five dots?
(A)
(B)
(C)
(D)
(E)
Grade 1-2
Year 2013
4. A lot of babies were born in the zoo last year: two baby lions, three baby dolphins and four baby eagles.
tulips. They started at 9:00 in the morning. At what time will they finish planting all 20 tulips?
(A) At 9:10
(B) At 9:20 (C) At 9:40
(D) At 9:50
(E) At 10:00
(C) 2 and 0
(D) 6 and 9
(E) 7 and 1
2. There are twelve books on a shelf and four children in a room. How
many books will be left on the shelf if each child takes one book?
(A) 16
(B) 30
(C) 50
(D) 52
(E) 70
18. In a park there are babies in four-wheel strollers and children on two-wheel bikes. Paula counted wheels and the total was 12. When she added the number of strollers to the number of bikes, the total was 4. How many two-wheel bikes are there in the park?
国际学校十年级数学期末测试卷(英语)

2. If cos q = 0.6691306, then q = a) 48° b) 42° c) 33.8° d) 22.9°
3. If DABC ~ DPQR, then a) AB = PQ b) A = P c) a = b d) c = r
Final Practice Exam
4. The cost of 4 L of milk is $7.35. The unit cost per litre is a) $3.68 b) $1.84 c) $2.45 d) $0.735
5. A 10% discount off an item priced at $30.00 would save you a) $3.00 b) $27.00 c) $10.00 d) $1.00
8. A rotation of a shape is a) a slide b) a translation c) a turn d) a mirror image
9. Which statement is true about angles with parallel lines with a transversal? a) All angles are 60° b) Interior alternate angles are complementary c) Corresponding angles are supplementary d) Exterior alternate angles are congruent
Final Practice Exam
3
4
Grade 10 Essential Mathematics
加拿大数学十年级(高一)省考模拟试题A

PART A: MULTIPLE-CHOICE QUESTIONS (calculator not permitted)
Value: 12 marks
Suggested Time: 30 minutes Allowable Time: 40 minutes
Page 1
Use the following graph to answer question 2.
y
10
5
–10
–5
–5
x
5
10
–10
2. Which of the following equations describes the linear relation graphed above?
Do not access your calculator until directed by the supervisor. At the end of the 40 minutes, the supervisor will give you permission to access your calculator.
where n is the number of students attending the prom. Calculate the cost of renting the hall if 70 students attend. A. $108 B. $500 C. $780 D. $970
6. Which of the following statements are true?
Contents: 31 pages 54 multiple-choice questions (maximum of 54 marks) 6 numeric-response questions (maximum of 6 marks)
加拿大安大略地区十学年数学试卷英文

GRADE 10 PRINCIPLES OF MATHEMATICS (ACADEMIC)MPM 2DTotal Marks:INSTRUCTIONS:1. Calculators may be used.2. Read all instructions carefully in order to maximize your mark.A/C[K] Part A – Multiple Choice 25 Marks (25 questions * 1 mark each)For each of the following questions in this section, circle the letterrepresenting the correct answer.1. A linear system of two equations that has one solution represents twolines that are:a) parallel b) coincident c) intersecting d) none of these2. The midpoint of RS is M(8, -1). If point S has coordinates (11, 4) what are the coordinates of point R ? a) (3, -6)b) (15, -6)c) (5, -6)d) (3, 9)3. The midpoint of the line segment with end points A(-8, 8) and B(6, 4) is: a) (0, 10)b) (1, 2)c) (7, 2)d) (-1, 6)4. The equation of a horizontal line passing through the point (4, 2) is: a) 2=xb) 4=yc) 2=yd) 4=x5. The equation of a line with a slope of 5=m and a y intercept of 8 is: a) 85+=x yb) 85+-=x yc) 85--=x yd) 58+=x y6. The slopes of 2 lines are -7 and 71. These lines are said to be:a) parallelb) perpendicularc) coincidentd) none of these7. The slope of a line segment passing through 2 points (10,- 4) and (-2, -16) is: a) 1b) 2c) -1d) -28. The length of a line segment with end points (-6, 7) and (-1, -5) is: a) 12b) 5c) 13d) 1699. The diameter of a circle whose equation is 28922=+y x is:a) 15b) 16c)17d) none of these10. T he equation of a circle with a centre of (0, 0) that also passes through the point (-8, -6) is: a) 1022=+y xb) 10022=+y xc) 1422=+y xd) 4822=-y x11. T he y-intercept of the line 01052=+-y x is: a) 2b) -2c) 10d) 512. T he slope of the line 0124=-+y x is: a) 2b) -2c) 1d) 013. I f (-3, y) is a solution to the equation 132=+y x , what is the value of y ? a) 3b) 6c) 5d) 814. T he product ()()z y x z y x 323243-- is equal to:a) 2612z xyb) 26412z y xc) 2612z xy -d) 00412z y x15. A simplified expression for ()()n m n m ----52 is: a) m 7b) n m 27+c) m 3-d) n m 27-16. A simplified expression for 242927abcbc a -- is:a) ac 3 b) abc 3 c) 23acd) 223c a17. T he slope of the line, which is perpendicula r to the line, 084=+-y x is: a) -4 b) 4 c) 1 d) -118. T he shortest distance from the point (2, -3) to the line 4-=x is: a) 5 b) 3 c) 2 d) 619. T he value of the polynomial 8542+-a a when 3-=a is: a) 59 b) 44 c) 13 d) 2920. W hich of the following is not a function : a)()()(){}7,6,5,4,3,2b) 22x y =c) 22y x =d) ()()(){}3,8,3,7,2,621. T he range of the relation whose equation is 52--=x y is: a) 5-≤y b) 5≤y c) 5-≥y d) 5≥y22. T he vertex of the parabola ()642--=x y is:a) ()6,4- b) ()6,4- c) ()4,6- d) ()4,6-23. T he equation of the axis of symmetry of the parabola ()5242+--=x y is:a) 5=x b) 5-=x c) 2=xd) 2-=x 24. A parabola with a vertex of ()3,2 and a stretch factor of 41- (relative to2x y =) would have an equation of: a) ()32412+--=x y b) ()32412++-=x y c)()23412-+-=x y d) ()23412++-=x y25 The parabola k x y +-=24 passes through the point ()3,2-. T he value of k is: a) -19b) 11c) 13d) 19A/CPart B – Short AnswersFor each of the questions in this section, write your answers in the spaces provided . Use the foolscap provided for any rough work. Show details of calculations wherever requested.1. In the accompanying diagram, state each of the following: (4 Marks) [K] a) domain: __________ (1 Mark)[K]b)range: __________ (1 Mark)[C]c)Is the relation a function? Justify your answer. (2 marks)[A] 2. The x-intercepts of the parabola 2892-y are: __________ and=x__________.(Show your work) (2 Marks)[A] 3. The roots of the quadratic equation 032=1710x are: __________ and+-x__________.(Show your work) (3 Marks)[A] 4. Write the equation of the parabola with a vertex of (4, 23) if it passesthrough the point (-1, -2): (Show your work) (3 Marks)____________________[T] 5. A line passes through 2 points (1, 4) and (2,-4). Calculate the slope of the line. Also show the equation of the line in the form 0ByAx. (Show+=+Cyour work) (4 Marks)____________________ ____________________Slope Equation[K] 6. The Tangent of 45 is: __________ (1 Mark)[A] 7. a) In the accompanying diagram, the two triangles are similar. What is thevalue of x?(Show your work) (2 Marks) Array=x__________[T] b) If the area of the smaller triangle is 8 cm 2, what is the area of the larger triangle?(Show your work) ( 2 Marks)Area = __________[K]8 Given that sin A = 21, find A ∠ (to the nearest degree) __________ (1Mark)[A] 9. In the accompanying right triangle , find the value of x to one decimal place.(Show your work) (2 Marks)=x ________[A] 10. U se the SINE LAW to find the value of side x to one decimal place.(Show your work) (2 Marks)3028︒x56︒42︒x30x = ________[A] 11. U se the COSINE LAW to find the value of side x to one decimal place.(Show your work) (2 Marks)x = ________[T] 12. F actor each of the following to the fullest extent possible: (4 Questions * 2 marks each)a) y x my mx 22--+________________________b) 31142--x x________________________c) 2416916y x -________________________56︒2030xd) 2225rs-________________________ r+9s30A/C Part C – Full Solutions RequiredFor each of the questions in this section, full solutions are required.Record your answers in the spaces provided. Use the foolscap providedfor any rough work.[A] 1. Solve the linear system using the elimination method. Remember tofind values for both x and y. (5 Marks)22+yx5=-yx=32-21[C] Explain what the solution above represents geometrically. How do youknow that the solution you arrived at is the correct answer? (2 Marks)[A]2. Expand and simplify the polynomial ()()()21432+-+-x x x . (4 Marks)[T]3. Find the equation of the line perpendicular to the line 088=-+y x and passing through the point (-4, 1). (4 Marks)[T] 4. From the window of one building, a man finds that the angle ofelevation to the top of a second building is 47︒ and the angle ofdepression to the bottom of the same building is 58︒. The buildings are 60 m apart. Find the height of the 2nd building to the nearest metre.A diagram is required. (6 Marks)[T] 5. ABC has vertices A(1, 7), B(-5, 3) and C(3, -1). Determine the equation for AE, the altitude from vertex A to the opposite side BC.(5 Marks)6. The hypotenuse of a right triangle is 26 cm. The sum of the other twosides is 34 cm. (9 Marks)[T] a) Find the length of the other two sides of the triangle. (3 Marks)[T] b) Find the measure of the other two angles. Round to the nearest degree. (3 Marks)[C] c) Describe a situation where you would be able to use knowledge ofthe Pythagorean theorem in a practical, real life situation. (3 Marks)[T] 7. A rectangular skating rink measures 20m by 20m. It has been decided to increase the area of the rink by a factor of 4. Determine how mucheach side should be extended. Assume that each side is extendedby the same amount. (6 Marks)[C]What is the significance of keeping the skating rink in the shape of a square? Justify your answer. (3 Marks)[A]8. a) Solve 35122+=d d using the quadratic formula. (2 Marks)[A] b) Solve 03122=-x by factoring. Check your solutions. (2 Marks)。
加拿大安大略省十年级数学试卷-英文

GRADE 10 PRINCIPLES OF MATHEMATICS (ACADEMIC)MPM 2DTotal Marks:INSTRUCTIONS:1. Calculators may be used.2. Read all instructions carefully in order to maximize your mark.A/C [K] Part A – Multiple Choice 25 Marks (25 questions * 1 mark each)For each of the following questions in this section, circle the letter representing the correct answer.1. A linear system of two equations that has one solution represents two lines that are:a) parallel b) coincident c) intersecting d) none of these2. The midpoint of RS is M(8, -1). If point S has coordinates (11, 4) what are the coordinates of point R ?a) (3, -6) b) (15, -6) c) (5, -6) d) (3, 9)3. The midpoint of the line segment with end points A(-8, 8) and B(6, 4) is:a) (0, 10) b) (1, 2) c) (7, 2) d) (-1, 6)4. The equation of a horizontal line passing through the point (4, 2) is:a) 2=x b) 4=y c) 2=y d) 4=x5. The equation of a line with a slope of 5=m and a y intercept of 8 is:a) 85+=x y b) 85+-=x y c) 85--=x y d) 58+=x y6. The slopes of 2 lines are -7 and71. These lines are said to be:a) parallel b) perpendicular c) coincident d) none of these7. The slope of a line segment passing through 2 points (10,- 4) and (-2, -16) is:a) 1 b) 2 c) -1 d) -28. The length of a line segment with end points (-6, 7) and (-1, -5) is:a) 12 b) 5 c) 13 d) 1699. The diameter of a circle whose equation is 28922=+y x is:a) 15 b) 16 c)17 d) none of these10. The equation of a circle with a centre of (0, 0) that also passes through the point (-8, -6) is:a) 1022=+y x b) 10022=+y x c) 1422=+y x d) 4822=-y x11. The y-intercept of the line 01052=+-y x is:a) 2 b) -2 c) 10 d) 512. The slope of the line 0124=-+y x is:a) 2 b) -2 c) 1 d) 013. If (-3, y) is a solution to the equation 132=+y x , what is the value of y ?a) 3 b) 6 c) 5 d) 814. The product ()()z y x z y x 323243-- is equal to:a) 2612z xy b) 26412z y x c) 2612z xy - d) 00412z y x15. A simplified expression for ()()n m n m ----52 is:a) m 7 b) n m 27+ c) m 3- d) n m 27-16. A simplified expression for 242927abcbc a -- is:a) ac 3 b) abc 3 c) 23ac d) 223c a17. The slope of the line, which is perpendicula r to the line, 084=+-y x is:a) -4 b) 4 c) 1 d) -118. The shortest distance from the point (2, -3) to the line 4-=x is:a) 5 b) 3 c) 2 d) 619. The value of the polynomial 8542+-a a when 3-=a is:a) 59 b) 44 c) 13 d) 2920. Which of the following is not a function :a) ()()(){}7,6,5,4,3,2 b) 22x y = c) 22y x = d) ()()(){}3,8,3,7,2,621. The range of the relation whose equation is 52--=x y is:a) 5-≤y b) 5≤y c) 5-≥y d) 5≥y22. The vertex of the parabola ()642--=x y is:a) ()6,4- b) ()6,4- c) ()4,6- d) ()4,6-23. The equation of the axis of symmetry of the parabola ()5242+--=x y is:a) 5=x b) 5-=x c) 2=x d) 2-=x24. A parabola with a vertex of ()3,2 and a stretch factor of 41- (relative to 2x y =) wouldhave an equation of:a) ()32412+--=x y b) ()32412++-=x y c)()23412-+-=x y d) ()23412++-=x y25 The parabola k x y +-=24 passes through the point ()3,2-. T he value of k is:a) -19b) 11c) 13d) 19A/CPart B – Short AnswersFor each of the questions in this section, write your answers in the spaces provided . Use the foolscap provided for any rough work. Show details of calculations wherever requested.1. In the accompanying diagram, state each of the following: (4 Marks)[K] a) domain: __________ (1 Mark) [K] b) range: __________ (1 Mark)[C]c) Is the relation a function? Justifyyour answer. (2 marks)[A] 2. The x-intercepts of the parabola 2892-=x y are: __________ and __________. (Show your work) (2 Marks)[A] 3. The roots of the quadratic equation 0101732=+-x x are: __________ and __________. (Show your work) (3 Marks)[A]4. Write the equation of the parabola with a vertex of (4, 23) if it passes through the point (-1, -2): (Show your work) (3 Marks)____________________ [T] 5. A line passes through 2 points (1, 4) and (2,-4). Calculate the slope of the line. Also show the equation of the line in the form 0=++CByAx. (Show your work) (4 Marks)____________________ ____________________Slope Equation[K] 6. The Tangent of45 is: __________ (1 Mark)[A] 7. a) In the accompanying diagram, the two triangles are similar. What is the value of x?(Show your work) (2 Marks)=x__________[T] b) If the area of the smaller triangle is 8 cm2, what is the area of the larger triangle?(Show your work) ( 2 Marks)Area = __________[K] 8 Given that sin A =21, find A∠ (to the nearest degree) __________ (1 Mark)[A] 9. In the accompanying right triangle, find the value of x to one decimal place.(Show your work) (2 Marks)=x ________[A] 10. Use the SINE LAW to find the value of side x to one decimal place. (Show your work) (2 Marks)x = ________[A] 11. Use the COSINE LAW to find the value of side x to one decimal place. (Show your work) (2 Marks)x = ________[T] 12. Factor each of the following to the fullest extent possible: (4 Questions * 2 marks each)a) y x my mx 22--+________________________b) 31142--x x________________________c) 2416916y x -________________________3028︒x56︒42︒x3056︒2030xd) 2225309s rs r +-________________________A/CPart C – Full Solutions RequiredFor each of the questions in this section, full solutions are required. Record your answers in the spaces provided. Use the foolscap provided for any rough work.[A] 1. Solve the linear system using the elimination method . Remember to find values for both x and y. (5 Marks) 225=+y x2132-=-y x[C]Explain what the solution above represents geometrically. How do you know that the solution you arrived at is the correct answer? (2 Marks)[A] 2. Expand and simplify the polynomial ()()()21432+-+-x x x . (4 Marks)[T] 3. Find the equation of the line perpendicular to the line 088=-+y x and passing through the point (-4, 1). (4 Marks)[T] 4. From the window of one building, a man finds that the angle of elevation to the top ofa second building is 47︒ and the angle of depression to the bottom of the samebuilding is 58︒. The buildings are 60 m apart. Find the height of the 2nd building tothe nearest metre. A diagram is required. (6 Marks)[T] 5. ∆ABC has vertices A(1, 7), B(-5, 3) and C(3, -1). Determine the equation for AE, the altitude from vertex A to the opposite side BC. (5 Marks)6. The hypotenuse of a right triangle is 26 cm. The sum of the other two sides is 34 cm.(9 Marks)[T] a) Find the length of the other two sides of the triangle. (3 Marks)[T] b) Find the measure of the other two angles. Round to the nearest degree. (3 Marks) [C] c) Describe a situation where you would be able to use knowledge of thePythagorean theorem in a practical, real life situation. (3 Marks)[T] 7. A rectangular skating rink measures 20m by 20m. It has been decided to increase the area of the rink by a factor of 4. Determine how much each side should beextended. Assume that each side is extended by the same amount. (6 Marks)[C]What is the significance of keeping the skating rink in the shape of a square? Justify your answer. (3 Marks)[A] 8. a) Solve 35122+=d d using the quadratic formula. (2 Marks)[A]b) Solve 03122=-x by factoring. Check your solutions. (2 Marks)。
加拿大中考数学试卷真题

一、选择题(每题4分,共40分)1. 下列各数中,有理数是()A. √2B. √-1C. πD. 0.333...2. 已知等差数列的前三项分别为2,5,8,则该数列的公差是()A. 3B. 4C. 5D. 63. 若一个三角形的三个内角分别为30°,60°,90°,则该三角形是()A. 直角三角形B. 锐角三角形C. 钝角三角形D. 等腰三角形4. 已知一次函数y=kx+b的图象经过点A(1,2)和B(3,4),则该函数的斜率k和截距b分别是()A. k=1,b=1B. k=1,b=2C. k=2,b=1D. k=2,b=25. 在平面直角坐标系中,点P(-2,3)关于原点的对称点是()A. (2,-3)B. (-2,-3)C. (-2,3)D. (2,3)6. 下列函数中,是反比例函数的是()A. y=x^2B. y=x^3C. y=1/xD. y=√x7. 若等比数列的首项为2,公比为3,则该数列的前5项之和为()A. 31B. 32C. 33D. 348. 在平面直角坐标系中,直线y=2x+1与y轴的交点坐标是()A. (0,1)B. (1,0)C. (-1,0)D. (0,-1)9. 若等差数列的前三项分别为3,8,13,则该数列的第四项是()A. 18B. 19C. 20D. 2110. 下列图形中,是轴对称图形的是()A. 正方形B. 长方形C. 梯形D. 平行四边形二、填空题(每题5分,共25分)11. 已知等差数列的前三项分别为a,a+d,a+2d,则该数列的通项公式为______。
12. 若一个三角形的两个内角分别为45°,135°,则该三角形的第三个内角是______。
13. 在平面直角坐标系中,点A(-3,2)关于x轴的对称点是______。
14. 若一次函数y=kx+b的图象经过点C(0,3),则该函数的截距b是______。
加拿大国际袋鼠数学竞赛试题-2013年

加拿⼤国际袋⿏数学竞赛试题-2013年Grade 1-2International Contest-Game MATH KANGAROOPart A: Each correct answer is worth 3 points. 1. Which digits are missing?Year 2013(A) 3 and 5 (B) 4 and 8(C) 2 and 0(D) 6 and 9(E) 7 and 12. There are twelve books on a shelf and four children in a room. Howmany books will be left on the shelf if each child takes one book?(A) 12(B) 8(C) 4(D) 2(E) 03. Which of the dresses has less than seven dots, but more than five dots?(A)(B)(C)(D)(E)Grade 1-2Year 20134. A lot of babies were born in the zoo last year: two baby lions, three baby dolphins and four baby eagles. How many legs do all these babies have altogether?(A) 20(B) 18(C) 16(D) 14(E) 125. Several students want to plant 20 tulips in the school garden. It takes ten minutes for them to plant five tulips. They started at 9:00 in the morning. At what time will they finish planting all 20 tulips?(A) At 9:10(B) At 9:20 (C) At 9:40(D) At 9:50(E) At 10:006. How many more bricks are there in the larger stack?(A) 4(B) 5(C) 6(D) 7Part B: Each correct answer is worth 4 points.(E) 107. Ann has. Barb gave Eve. Jim has. Bob has. Who is Barb?(A)(B)(C)8. There is a path with square tiles.(D)(E)How many tiles fit in the area inside?(A) 5(B) 6(E) 9Grade 1-2Year 20139. Cat and Mouse are moving to the right. When Mouse jumps 1 tile, Cat jumps 2 tiles at the same time.On which tile does Cat catch Mouse?(A) 1(B) 2(C) 3(D) 4(E) 510. I am a number. If you count by tens you will say my name. I am not ten. If you add me to 30, you will get anumber less than 60. Who am I?(A) 20(B) 30(C) 40(D) 50(E) 6011. There is a house on each corner of the streets. The housesare shown on the map. Two new houses will be built oneach street between the corner houses. How many houseswill there be in all?(A) 8(B) 12(C) 16(D) 20(E) Other answer12. Kasia has 3 brothers and 3 sisters. How many brothers and how many sisters does her brother Mike have?(A) 3 brothers and 3 sisters(B) 3 brothers and 4 sisters(C) 2 brothers and 3 sisters(D) 3 brothers and 2 sisters(E) 2 brothers and 4 sistersPart C: Each correct answer is worth 5 points.13. Ania makes a large cube from 27 small white cubes. She paints all the faces of the large cube. Then Ania removes four small cubes from four of the corners, as shown. While the paint is still wet, she stamps each of the new faces onto a piece of paper. How many of the following stamps can Ania make?(A) 1(B) 2(C) 3(D) 4(E) 514. Ann has a lot of these pieces:She tries to put them in the square, as many as possible. How many cells shall be left empty?(A) 0(B) 1(C) 2(D) 3(E) 4Grade 1-215. In a game it is possible to make the following exchanges:Year 2013Adam has 6 pears. How many strawberries will Adam have, when he trades all his pears for juststrawberries?(A) 12(B) 36(C) 1816. Sophie makes a row of 10 houses with matchsticks. In the picture you can see the beginning of the row. How many matchsticks does Sophie need altogether?(A) 50(B) 51(C) 55(D) 60(E) 6217. A square box is filled with two layers of identical square pieces of chocolate. Kirill has eaten all 20 pieces in the upper layer, which are along the walls of the box. How many pieces of chocolate are left in the box?(A) 16(B) 30(C) 50(D) 52(E) 7018. In a park there are babies in four-wheel strollers and children on two-wheel bikes. Paula counted wheels and the total was 12. When she added the number of strollers to the number of bikes, the total was 4. How many two-wheel bikes are there in the park?(A) 1(B) 2(C) 3(D) 4(E) Other numberGrade 3-4Year 2013International Contest-Game MATH KANGAROOPart A: Each correct answer is worth 3 points. 1. In which figure is the number of black kangaroos bigger than the number of white kangaroos?(A)(B)(C)(D)(E)2. Aline writes a correct calculation. Then she covers two digits which are the same with a sticker:Which digit is under the stickers?(A)(B)(C)(D)(E)3. Monica arrived in the Kangaroo Camp on July 25th in the morning and left the camp on August 3rd inthe afternoon. How many nights did she sleep in the camp?(A) 7(B) 9(C) 10(D) 30(E) 84. How many triangles of all sizes can be seen in the picture below?(A) 9(B) 10(C) 11(D) 13(E) 125. In London 2012, the USA won the most medals: 46 gold, 29 silver and 29 bronze. China was secondwith 38 gold, 27 silver and 23 bronze. How many more medals did the USA win compared to China?(A) 6(B) 14(C) 16(D) 24Grade 3-4Year 20136. There are three families in my neighbourhood with three children each; two of the families havetwins. All twins are boys. At most how many girls are in these families?(A) 2(B) 3(C) 4(D) 5(E) 67. Vero's mother prepares sandwiches with two slices of bread each. A package of bread has 24 slices.How many sandwiches can she prepare from two and a half packages of bread?(A) 24(B) 30(C) 48(D) 34(E) 268. About the number 325, five boys said:Andrei: "This is a 3-digit number"Boris: "All digits are distinct"Vick: "The sum of the digits is 10"Greg: "The units digit is 5"Danny: "All digits are odd"Which of the boys was wrong?(A) Andrei(B) Boris(C) Vick(D) Greg(E) DannyPart B: Each correct answer is worth 4 points. 9. The rectangular mirror was broken.Which of the following pieces is the missing part of the broken mirror?(A)(B)(C)(D)(E)10. When Pinocchio lies, his nose gets 6 cm longer. When he tells the truth, his nose gets 2 cm shorter. When his nose was 9 cm long, he told three lies and made two true statements. How long was Pinocchio's nose afterwards?(A) 14 cm(B) 15 cm(C) 19 cm(D) 23 cm(E) 31 cmGrade 3-4Year 201311. John is 33 years old. His three sons are 5, 6 and 10 years old. In how many years will the three sons together be as old as their father?(A) 4(B) 6(C) 8(D) 10(E) 1212. On the map, white lines represent streets. There are pictograms on some intersections (for example, trafic light, basket, tram). Ann started walking at the beginning of the middle vertical street in the direction of the arrow. At every intersection of streets she turned either to the right or to the left. First she turned right, then left, then again left, then right, then left, and finally again left. Which of the landmarks did Ann approach in the end?(A)(B)(E)13. Schoolmates Andy, Betty, Cathie and Dannie were born in the same year. Their birthdays were on February 20th, April 12th, May 12th and May 25th, not necessarily in this order. Betty and Andy were born in the same month. Andy and Cathie were born in the same day of different months. Who of these schoolmates is the oldest?(A) Andy(B) Betty(C) Cathie (D) Dannie (E) impossible to determine14. In the Adventure Park, 30 children took part in two of the adventures. 15 of them participated in the "moving bridge" contest, and 20 of them went down the zip-wire. How many of the children took part in both adventures?(A) 25(B) 15(C) 30(D) 10(E) 515. Which of the five pieces in the answers fits with the piece in the separate picture, so that together they form a rectangle?(A)(B)(C)(D)(E)16. Children in the school club had to arrange fitness balls according to their size from the biggest to the smallest one. Rebecca was comparing them and said: the red ball is smaller than the blue one, the yellow one is bigger than the green one, and the green one is bigger than the blue one. What is the correct order of the fitness balls?(A) green, yellow, blue, red (D) yellow, green, blue, red(B) red, blue, yellow, green (E) blue, yellow, green, red(C) yellow, green, red, blueGrade 3-4Year 2013Part C: Each correct answer is worth 5 points.17. In the shown triangle, first we join the midpoints of all the three sides. This way, we form a smaller triangle. We repeat this one more time with the smaller triangle, forming a new even smaller triangle, which we colour in red. How many triangles of the size of the red triangle are needed to cover completely the original triangle, without overlapping?Note: Midpoint of a side is the point that divides the side in two parts of the same length.(A) 5(B) 8(C) 10(D) 16(E) 3218. There are oranges, apricots and peaches in a big basket. How many fruits are there in the basket if the peaches and the apricots together are 18, the oranges and the apricots together are 28 and 30 fruits are not apricots?(A) 46(B) 20(C) 40(D) 38(E) 2919. In December Tom-the-cat slept for exactly 3 weeks. Which calculations should we do in order to find how many minutes he stayed awake during this month?(A) (31 – 7) × 3 × 24 × 60(B) (31 – 7 × 3) × 24 × 60(C) (30 – 7 × 3) × 24 × 60(D) (31 – 7 ) × 24 × 60(E) (31 – 7 × 3) × 24 × 60 × 6020. Basil has several domino tiles, as shown in the figure. He wants to arrange them in a line according to the well-known "domino rule": in any two tiles that are next to each other, the squares that touch must have the same number of points. What is the largest number of tiles he can arrange in this way?(A) 3(B) 4(C) 521. Cristi has to sell 10 glass bells that vary in price: 1 euro, 2 euro, 3 euro, 4 euro, 5 euro, 6 euro, 7 euro, 8 euro, 9 euro, 10 euro. In how many ways can Cristi divide all the glass bells in three packages so that all the packages have the same price?(A) 1(B) 2(C) 3(D) 4(E) Such a division is not possible.Grade 3-4Year 201322. Nancy bought 17 cones of ice-cream for her three children. Misha ate twice as many cones as Ana. Dan ate more ice-cream than Ana but less than Misha. How many cones of ice-cream did Dan eat?(A) 4(B) 5(C) 6(D) 7(E) 823. Peter bought a carpet 36 dm wide and 60 dm long. The figure shows part of this carpet. As seen, the carpet has a pattern of small squares containing either a sun or a moon. You can count that along the width there are nine squares. When the carpet is fully unrolled, how many moons will be seen?(A) 68(B) 67(C) 65(D) 63(E) 6024. Beatrice has a lot of pieces like the grey one in the picture. At least how many of these grey pieces will she need to makea grey square?(A) 3(B) 4(C) 6(D) 8(E) 16Grade 11-12International Contest-Game MATH KANGAROOPart A: Each correct answer is worth 3 points.Year 20131. Which of the following numbers is the largest?(A) 2013(B) 20+13(C) 2013(D) 2013(E) 20 × 132. Four circles of radius 1 are touching each other and a smaller circle as seen in the picture. What is the radius of the smaller circle?(A) 2 ?11 (B)23 (C)43 (D)47 (E)163. A three-dimensional object bounded only by polygons is called a polyhedron. What is the smallestnumber of polygons that can bind a polyhedron, if we know that one of the polygons has 12 sides?(A) 12(B) 13(E) 244. The cube root of 333 is equal to(A) 33(B) 333 ?1(C) 323(D) 332(E) ( 3)35. The year 2013 has the property that its number is made up of the consecutive digits 0, 1, 2 and 3.How many years have passed since the last time a year was made up of four consecutive digits?(A) 467(B) 527(C) 581(D) 693(E) 9906. Let f be a linear function for which f(2013) – f(2001) = 100. What is f(2031) – f(2013)?(A) 75(B) 100(C) 120(D) 150(E) 1807. Given that 2 < x < 3, how many of the following statements are true?4 < x2 < 94 < 2x < 96 < 3x < 9 0 < x2 ? 2x < 3(A) 0(B) 1(C) 2(D) 3(E) 48. Six superheroes capture 20 villains. The first superhero captures one villain, the second capturestwo villains and the third captures three villains. The fourth superhero captures more villains thanany of the other five. What is the smallest number of villains the fourth superhero must havecaptured?(A) 7(B) 6(C) 5(D) 4(E) 3Grade 11-12Year 20139. In the cube to the right you see a solid, non-transparent pyramid ABCDS with base ABCD, whose vertex S lies exactly in the middle of an edge of the cube. You look at this pyramid from above, from below, from behind, from ahead, from the right and from the left. Which view does not arise?(A)(B)(C)(D)(E)10.Whena certainsolid substancemelts,itsvolume increasesby1 12.By how much doesitsvolumedecrease when it solidifies again?(A)1 10(B)1 11(C)1 12(D)1 13(E)1 14Part B: Each correct answer is worth 4 points.11. The diagram shows two squares of equal side length placed so thatthey overlap. The squares have a common vertex and the sides make anangle of 45 degrees with each other, as shown. What is the area of theoverlap as a fraction of the area of one square?1 (A)21 (B)2(C) 1? 1 2(D) 2 ?12 ?1 (E)212.How many positive integers n exist such that bothn 3and 3nare three-digit integers?(A) 12(B) 33(C) 34(D) 100(E) 30013. A circular carpet is placed on a floor of square tiles. All the tiles which have more than one point in common with the carpet are marked grey. Which of the following is an impossible outcome?(A)(B)(C)(D)(E)14. Consider the following statement about a function f on the set of integers: "For any even x, f(x) is even." What would be the negation of this proposition?(A) For any even x, f(x) is odd(B) For any odd x, f(x) is even(C) For any odd x, f(x) is odd(D) There exists an even number x such that f(x) is odd(E) There exists an odd number x such that f(x) is oddGrade 11-12Year 201315. How many pairs (x,y) of positive integers satisfy the equation x2 y3 = 612 ?(A) 6(B) 8(C) 10(D) 12(E) Another number.16. Given a function W (x) = (a ? x)(b ? x)2 , where a < b. Its graph is in one of the following figures. In which one?(A)(B)(C)(D)(E)17. Consider a rectangle, one of whose sides has a length of 5. The rectangle can be cut into a squareand a rectangle, one of which has the area 4. How many such rectangles exist?(A) 1(B) 2(C) 3(D) 4(E) 518. Assume that x2 ? y2 = 84 , where x and y are positive integers. How many values may theexpression x2 + y2 have?(A) 1(B) 2(C) 3(D) 5(E) 619. In the triangle ABC the points M and N on the side AB are such that AN = ACand BM = BC. Find ∠ACB if ∠MCN = 43°.(A) 86°(B) 89°(C) 90°(D) 92°(E) 94°20. A box contains 900 cards numbered from 100 to 999. Any two cards have different numbers.Fran?ois picks some cards and determines the sum of the digits on each of them. At least how manycards must he pick in order to be certain to have three cards with the same sum?(A) 51(B) 52(C) 53(D) 54(E) 55Part C: Each correct answer is worth 5 points.21. How many pairs (x,y) of integers with x ≤ y exist such that their product equals 5 times their sum?(A) 4(B) 5(C) 6(D) 7(E) 822. Let f (x), x ∈ R be the function defined by the following properties: f is periodic with period 5 andf (x) = x2 when x ∈[?2,3) . What is f(2013) ?(A) 0(B) 1(C) 2(D) 4(E) 923. We have many white cubes and many black cubes, all of the same size. We want to build a rectangular prism composed by exactly 2013 of these cubes so that they are placed alternating a white cube and a black cube in all directions. If we start putting a black cube in one of the eight corners of the prism, how many black squares will we see on the exterior surface of the solid?(A) 887(B) 888(C) 890(E) It depends on the dimensions of the prism(D) 892Grade 11-12Year 201324. How many solutions (x,y), where x and y are real numbers, does the equation x2 + y2 = x + yhave? (A) 1(B) 5(C) 8(D) 9(E) Infinitely many.25. There are 2013 points marked inside a square. Some of them are connected to the vertices of thesquare and with each other so that the square is divided into non-overlapping triangles. All markedpoints are vertices of these triangles. How many triangles are formed this way?(A) 2013(B) 2015(C) 4026(D) 4028(E) impossible to determine26. There are some straight lines drawn on the plane. Line a intersects exactly three other lines and lineb intersects exactly four other lines. Linec intersects exactly n other lines, with n ≠ 3, 4 .Determine the number of lines drawn on the plane.(A) 4(B) 5(C) 6(D) 7(E) Another number.27. The sum of the first n positive integers is a three-digit number in which all of the digits are thesame. What is the sum of the digits of n?(A) 6(B) 9(C) 12(D) 15(E) 1828. On the island of Knights and Knaves there live only two types of people: Knights (who always speak the truth) and Knaves (who always lie). I met two men who lived there and asked the taller man if they were both Knights. He replied, but I could not figure out what they were, so I asked the shorter man if the taller was a Knight. He replied, and after that I knew which type they were. Were the men Knights or Knaves?(A) They were both Knights.(B) They were both Knaves.(C) The taller was a Knight and the shorter was a Knave.(D) The taller was a Knave and the shorter was a Knight.(E) Not enough information is given.29. Julian has written an algorithm in order to create a sequence of numbers as a1 = 1,am+n = am + an + mn , where m and n are natural numbers. Find the value of a100.(A) 100(B) 1000(C) 2012(D) 4950(E) 505030. The roundabout shown in the picture is entered by 5 cars at the same time, eachone from a different direction. Each of the cars drives less than one round and notwo cars leave the roundabout in the same direction. How many differentcombinations are there for the cars leaving the roundabout?(A) 24(B) 44(C) 60(D) 81(E) 120Year 2013Grade 1 and 2 DBACCB DEDABE DACBDBGrade 3 and 4 DDBBCDBE BDBADEBD DDBCEBBBGrade 5 and 6 ECCBEBBECD CCDBADDACD ADBABDBBDBGrade 7 and 8 DBACEECEAC DEBCBAABBC AEDCCABDBCGrade 9 and 10 DBCCBAECBC DBDADDBCEB DCCEEDCCBBGrade 11 and 12 CABDCDEBED DAEDEADBEC ADCED*CBDEB*Answer E was also accepted as correct for Q25 Answers。
加拿大国际袋鼠数学竞赛试题 -2008年
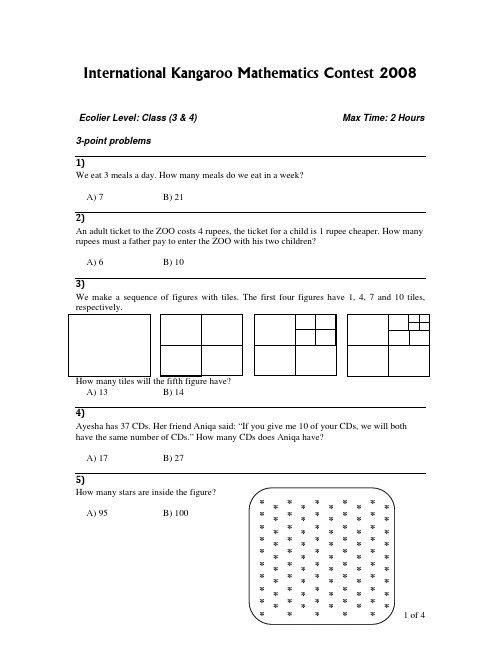
International Kangaroo Mathematics Contest 2008Ecolier Level: Class (3 & 4)Max Time: 2 Hours3-point problems1)We eat 3 meals a day. How many meals do we eat in a week?A) 7 B) 212)An adult ticket to the ZOO costs 4 rupees, the ticket for a child is 1 rupee cheaper. How many rupees must a father pay to enter the ZOO with his two children?A) 6 B) 10 3)We make a sequence of figures with tiles. The first four figures have 1, 4, 7 and 10 tiles, respectively.How many tiles will the fifth figure have? A) 13 B) 144)Ayesha has 37 CDs. Her friend Aniqa said: “If you give me 10 of your CDs, we will both have the same number of CDs.” How many CDs does Aniqa have?A) 17 B) 27 5)How many stars are inside the figure?A) 95 B) 100Rabia has drawn a point on a piece of paper. She now draws four straight lines that pass through this point. Into how many sections do these lines divide the paper?A) 4 B) 87)In six and one half hours it will be four hours after midnight. What time is it now?A) 21:30 B) 10:308)The storm made a hole in the front side of the roof. There were 10 roof tiles in each of 7 rows. How many tiles are left on the front side of the roof?A) 57 B) 599)Ejaz is making figures with two triangular cards shown. Which figure he cannot get?A) B)Ahmad multiplies by 3, Nasir adds 2, and Tahir subtracts 1. In what order can they do this to convert 3 into 14?A) Ahmad, Nasir, Tahir B) Nasir, Ahmad, Tahir11)Usman is taller than Noman and shorter than Salman. Who is the tallest?A) Usman B) Salman12)Abida made the figure on the right out of five cubes. Which ofthe following figures (when seen from any direction) can shenot get from the figure on the right side if she is allowed tomove exactly one cube?A) B)13)Which of the following figures is shown most often in the above sequence?B) All of them are shown equally often14)In a hotel, how many two-bed rooms should be added to 5 three-bed rooms to host 21 guests?A) 3 B) 615)There are three songs on a CD. The first song is 6 minutes and 25 seconds long, the second song is 12 minutes and 25 seconds long, and the third song is 10 minutes and 13 seconds long. How long are all the three songs together?A) 29 minutes 3 seconds B) 31 minutes 13 seconds16)We have a large number of blocks of 1 x 2 x 4 cm. We will try to put as many of these blocks as possible in a box of 4 x 4 x 4 cm so that we can close the box with a lid. How many blocks fit in?A) 8 B) 1017)Shaheen shoots two arrows at the target. In the drawing we see that her score is 5. If both arrows hit the target, how many different scores can she obtain?A) 6 B) 318)A garden in the shape of a square is divided into a pool (P) a flowerbed (F) a lawn (L) and a sandpit (S) (see the picture). The lawn and the flowerbed are in the shape of a square. The perimeter of the lawn is 20 m, the perimeter of the flowerbed is 12 m. What is the perimeter of the pool?A) 12 m B) 16 m19)Zahid has as many brothers as sisters. His sister Zahida has twice as many brothers as she has sisters. How many children are there in this family?A) 3 B) 7 20)How many two-digit numbers are there in which the digit on the right is larger than the digit on the left?A) 26 B) 36_______________________________GOOD LUCK !。
加拿大数学10年级练习第四部分几何

1. Find the ratio of the perimeter of the larger rectangle to the perimeter of the smaller rectangle.••••2. Find the area of the triangle with ∠A = 41, b = 5 ft, and c = 4 ft. Round your answer to two decimal places.• 6.56 ft2•10.00 ft2•7.55 ft2•13.12 ft23. Find the area of kite ABCD if BD = 48 cm, AB = 25 cm, and BC = 26. The kite is not drawn to scale.•289 cm2•70 cm2•816 cm2•408 cm24. The diameter of a basketball rim is 18 inches. A standard basketball has a circumference of 30 inches. Abouthow much room is there between the ball and the rim in a shot in which the ball goes in exactly in the center of the rim?•none of these5. Find the area.•718 square units•545 square units•534.5 square units•701 square units6. Name the major arc and find its measure.•m = 275•m = 170•m = 85•m = 2757. Find the area of the regular polygon. Round your answer to the nearest tenth.•110.2 in.28. Name the minor arc and find its measure.•m = 275•m = 85•m = 170•m = 2759. Find the area of ΔABC. The figure is not drawn to scale.•26.06 cm2•28.00 cm2•22.73 cm2•24.95 cm210. Find the circumference of the circle. Use as an approximation of π.•11 cm•5 cm•6 cm11. If a dart hits the target at random, what it the probability that it will land in the unshaded region?••••12. Find the area of the circle. Use π = 3.14 and round to the nearest hundredth.•91.56 m2•16.96 m2• 5.72 m2•22.89 m213. Find the area of a regular pentagon with side 6 cm.•45.2 cm2•123.9 cm214. Find the probability that an object falling randomly on the figure will land in the shaded area.•0.32•0.36•0.5•0.2615. Find the area.•102.9 yd2•205.8 yd2•35.35 yd2•105.5 yd216. Two concentric circles have radii of 14 cm and 24 cm. Find the probability to the nearest thousandth that apoint chosen at random from the circles is located outside the smaller circle and inside the larger one.•0.066•0.58317. A slide that is inches by inches is projected onto a screen that is 3 feet by 7 feet, filling the screen.What will be the ratio of the area of the slide to its image on the screen?• 1 : 112• 1 : 2304• 2 : 4205• 1 : 12,54418. Find the area of a regular octagon with perimeter 48 cm.•188.1 cm2•190.5 cm2•347.6 cm2•173.8 cm219. Dorothy ran 6 times around a circular track that has a diameter of 47 m. Approximately how far did she run?Use π = 3.14 and round your answer to the nearest meter.•885 m•1328 m•443 m•1734 m20. Find the area.•10.26 cm21. Find the volume of the cylinder in terms of π.•24π in.3•48π in.3•56π in.3•288π in.32. Find the volume of the cylinder in terms of π.•287π in.3•275π in.2•275π in.3•287π in.23. A sphere has a volume of 288π ft3. Find the surface area of the sphere.•864π ft2•48π ft2•144π ft2•96π ft24. The volumes of two similar solids are 2197 m3 and 64 m3. The surface area of the larger one is 845 m2. What is•64 m2•320 m2•80 m2•none of these5. Use a net to find the surface area of the prism.•114 m2•240 m2•290 m2•145 m26. Find the surface area of a sphere that has a diameter of 4 cm.•64π cm2•π cm3•4π cm2•16π cm27. Find the lateral area and the surface area of the cone. Use 3.14 for πand round the answer to the nearest hundredth.The diagram is not to scale.•lateral area: 733.33 ft2; surface area: 690.80 ft2•lateral area: 690.80 ft2; surface area: 1004.80 ft2•none of these8. If the ratio of the radii of two spheres is 7 : 2, what is the ratio of the surface areas of the two spheres?•7 : 2•7r2π : 2r2π•49 : 4•343 : 89. Use a net to find the surface area of the prism.•465 m2•720 m2•918 m2•930 m210. Find the height of the cylinder to the nearest tenth of an inch.•94.6 in.•96.6 in.• 4.3 in.• 4.1 in.11. Use formulas to find the lateral area and the surface area of the prism. Show your answer to the nearest hundredth.•63.00 m2; 567.00 m2•36.00 m2; 1134.00 m2•479.22 m2; 533.22 m2•542.22 m2; 596.22 m212. Find the volume of the prism.•942 m3•38 m3•945 m3•1890 m313. The volumes of two similar solids are 1331 m3 and 343 m3. The surface area of the larger one is 484 m2. Whatis the surface area of the smaller one?•343 m2•1372 m2•196 m2•none of these14. Find the surface area of the sphere.•648π m2•72π m2•324π m2•1296π m215. Find the volume of the prism.•40.5 m3•162 m3•9 m3•81 m316. Find the surface area of the solid. Round to the nearest square foot.•36 ft2•68 ft217. Use formulas to find the surface area of the prism. Show your answer to the nearest hundredth.•75.42 cm2•170.16 cm2•86.94 cm2•69.52 cm218. Which figure is a net for a cube?••••19. Cylinder A has radius 1 and height 4 and cylinder B has radius 2 and height 4. Find the ratio of the volumesof the two cylinders.• 1 : 4• 1 : 120. Neil had a job helping a jeweler. He had the assignment of counting the faces, vertices, and edges on the emeralds.On the first emerald, Neil counted 9 faces and 16 edges. He quickly realized he didn't have to count the vertices.How many vertices were there?•10 vertices•7 vertices•8 vertices•9 vertices1. Find the value of x if AB = 20, BC = 12, and CD = 13. (not drawn to scale)•18.8•16.5•13.4•14.92. Find the measure of each variable if m∠A = 22 and m = 97. (not drawn to scale)•53; 210•53; 105•75; 210•75; 1053. In the plane of lines X and Y, what is the locus of points equidistant from lines X and Y?•line A•line D•line B•line C4. A small messenger company can only deliver within a certain distance from the company. On the graph below, thecircular region represents that part of the city where the company delivers, and the center of the circle represents the location of the company. Which equation represents the boundary for the region where the company delivers?•(x + 3)2 + (y– 1)2 = 49•(x + 3)2 + (y– 3)2 = 98•(x + 1)2 + (y– 3)2 = 98•(x + 3)2 + (y– 3)2 = 495. A low-watt radio station can be heard only within a certain distance from the station. On the graph below, thecircular region represents that part of the city where the station can be heard, and the center of the circle represents the location of the station. Which equation represents the boundary for the region where the station can be heard?•(x– 4)2 + (y– 5)2 = 50•(x + 5)2 + (y– 5)2 = 59•(x + 5)2 + (y + 4)2 = 25•(x + 5)2 + (y– 5)2 = 256. Find the center and radius of (x– 8)2 + (y + 7)2 = 64.•(–8, 7); 8•(–7, 8); 64•(8, –7); 8•(–7, –8); 87. is tangent to circle O at B. How close to the circle is point A? (The diagram is not to scale.)• 3• 4.5• 6•7.58. Compare the quantity in Column A with the quantity in Column B.•The quantity in Column A is greater.•The quantity in Column B is greater.•The two quantities are equal.•The relationship cannot be determined from the information given.9. Solve for x.•22•710. Find the value of x.•14.6•8.1•9.4•13.411. Write the standard equation for the circle with center (14, –48) that passes through (0, 0).•(x– 14)2 + (y + 48)2 = 2500•(x + 14)2 + (y– 48)2 = 2500•(x + 14)2 + (y– 48)2 = 50•(x– 14)2 + (y + 48)2 = 5012. Find the measure of ∠BAC.•30°•150°13. Find the value of x.•79•39•99•15914. In space, which description fits the locus of points 3 cm from ?•an open cylinder of diameter 6 cm•an open cylinder of radius 3 cm and two hemispheres of diameter 6 cm each•an open cylinder of radius 3 cm and height 6 cm•an open cylinder of diameter 6 cm and two spheres of radius 3 cm each15. and are tangent to circle O and bisects ∠BPA. If m∠AOC= 68°, how much greater is m∠BCO thanm∠OAD? (The diagram is not to scale.)•22°•112°16. Write the standard equation for the circle with center (6, –8) that passes through (0, 0).•(x + 6)2 + (y– 8)2 = 0•(x– 6)2 + (y + 8)2 = 0•(x + 6)2 + (y– 8)2 = 100•(x– 6)2 + (y + 8)2 = 10017. Find the center and radius of (x + 3)2 + (y + 8)2 = 169.•(3, 8); 13•(–8, 3); 13•(–3, –8); 13•(–8, –3); 16918. , , and are all tangent to circle O. If JA= 8, AL= 13, and CK= 11, what is the perimeter of ΔJKL?(The diagram is not to scale.)•32•64•45•5319. If m = 38, what is m∠YAC?•109°•52°20. Write the standard equation for the circle with center (–16, 30) that passes through (0, 0).•(x + 16)2 + (y– 30)2 = 34•(x + 16)2 + (y– 30)2 = 1156•(x– 16)2 + (y + 30)2 = 1156•(x– 16)2 + (y + 30)2 = 34。
加拿大数学10年级练习第四部分几何电子教案

加拿大数学10年级练习第四部分几何1. Find the ratio of the perimeter of the larger rectangle to the perimeter of the smaller rectangle.••••2. Find the area of the triangle with ∠A = 41, b = 5 ft, and c = 4 ft. Round your answer to two decimalplaces.• 6.56 ft2•10.00 ft2•7.55 ft2•13.12 ft23. Find the area of kite ABCD if BD = 48 cm, AB = 25 cm, and BC = 26. The kite is not drawn to scale.•289 cm2•70 cm2•816 cm2•408 cm24. The diameter of a basketball rim is 18 inches. A standard basketball has a circumference of 30 inches.About how much room is there between the ball and the rim in a shot in which the ball goes in exactly in the center of the rim?• 4.2 in.• 4.78 in.•none of these5. Find the area.•718 square units•545 square units•534.5 square units•701 square units6. Name the major arc and find its measure.•m = 275•m = 170•m = 85•m = 2757. Find the area of the regular polygon. Round your answer to the nearest tenth.•40.0 in.2•67.6 in.2•110.2 in.28. Name the minor arc and find its measure.•m = 275•m = 85•m = 170•m = 2759. Find the area of ΔABC. The figure is not drawn to scale.•26.06 cm2•28.00 cm2•22.73 cm2•24.95 cm210. Find the circumference of the circle. Use as an approximation of π.•11 cm•5 cm•6 cm11. If a dart hits the target at random, what it the probability that it will land in the unshaded region?••••12. Find the area of the circle. Use π = 3.14 and round to the nearest hundredth.•91.56 m2•16.96 m2• 5.72 m2•22.89 m213. Find the area of a regular pentagon with side 6 cm.•76.6 cm2•45.2 cm2•123.9 cm214. Find the probability that an object falling randomly on the figure will land in the shaded area.•0.32•0.36•0.5•0.2615. Find the area.•102.9 yd2•205.8 yd2•35.35 yd2•105.5 yd216. Two concentric circles have radii of 14 cm and 24 cm. Find the probability to the nearest thousandth thata point chosen at random from the circles is located outside the smaller circle and inside the larger one.•0.066•0.583•0.01717. A slide that is inches by inches is projected onto a screen that is 3 feet by 7 feet, filling thescreen. What will be the ratio of the area of the slide to its image on the screen?• 1 : 112• 1 : 2304• 2 : 4205• 1 : 12,54418. Find the area of a regular octagon with perimeter 48 cm.•188.1 cm2•190.5 cm2•347.6 cm2•173.8 cm219. Dorothy ran 6 times around a circular track that has a diameter of 47 m. Approximately how far did she run?Use π = 3.14 and round your answer to the nearest meter.•885 m•1328 m•443 m•1734 m20. Find the area.•10.26 cm2•61.56 cm21. Find the volume of the cylinder in terms of π.•24π in.3•48π in.3•56π in.3•288π in.32. Find the volume of the cylinder in terms of π.•287π in.3•275π in.2•275π in.3•287π in.23. A sphere has a volume of 288π ft3. Find the surface area of the sphere.•864π ft2•48π ft2•144π ft2•96π ft24. The volumes of two similar solids are 2197 m3 and 64 m3. The surface area of the larger one is 845 m2. Whatis the surface area of the smaller one?•64 m2•320 m2•80 m2•none of these5. Use a net to find the surface area of the prism.•114 m2•240 m2•290 m2•145 m26. Find the surface area of a sphere that has a diameter of 4 cm.•64π cm2•π cm3•4π cm2•16π cm27. Find the lateral area and the surface area of the cone. Use 3.14 for π and round the answer to the nearesthundredth. The diagram is not to scale.•lateral area: 733.33 ft2; surface area: 690.80 ft2•lateral area: 6908.00 ft2; surface area: 1004.80 ft2•lateral area: 690.80 ft2; surface area: 1004.80 ft2•none of these8. If the ratio of the radii of two spheres is 7 : 2, what is the ratio of the surface areas of the twospheres?•7 : 2•7r2π : 2r2π•49 : 4•343 : 89. Use a net to find the surface area of the prism.•465 m2•720 m2•918 m2•930 m210. Find the height of the cylinder to the nearest tenth of an inch.•94.6 in.•96.6 in.• 4.3 in.• 4.1 in.11. Use formulas to find the lateral area and the surface area of the prism. Show your answer to the nearesthundredth.•63.00 m2; 567.00 m2•36.00 m2; 1134.00 m2•479.22 m2; 533.22 m2•542.22 m2; 596.22 m212. Find the volume of the prism.•942 m3•38 m3•945 m3•1890 m313. The volumes of two similar solids are 1331 m3 and 343 m3. The surface area of the larger one is 484 m2.What is the surface area of the smaller one?•343 m2•1372 m2•196 m2•none of these14. Find the surface area of the sphere.•648π m2•72π m2•324π m2•1296π m215. Find the volume of the prism.•40.5 m3•162 m3•9 m3•81 m316. Find the surface area of the solid. Round to the nearest square foot.•36 ft2•32 ft217. Use formulas to find the surface area of the prism. Show your answer to the nearest hundredth.•75.42 cm2•170.16 cm2•86.94 cm2•69.52 cm218. Which figure is a net for a cube?••••19. Cylinder A has radius 1 and height 4 and cylinder B has radius 2 and height 4. Find the ratio of thevolumes of the two cylinders.• 1 : 4• 5 : 620. Neil had a job helping a jeweler. He had the assignment of counting the faces, vertices, and edges on theemeralds. On the first emerald, Neil counted 9 faces and 16 edges. He quickly realized he didn't have to count the vertices. How many vertices were there?•10 vertices•7 vertices•8 vertices•9 vertices1. Find the value of x if AB = 20, BC = 12, and CD = 13. (not drawn to scale)•18.8•16.5•13.4•14.92. Find the measure of each variable if m∠A = 22 and m = 97. (not drawn to scale)•53; 210•53; 105•75; 210•75; 1053. In the plane of lines X and Y, what is the locus of points equidistant from lines X and Y?•line A•line D•line B•line C4. A small messenger company can only deliver within a certain distance from the company. On the graph below,the circular region represents that part of the city where the company delivers, and the center of the circle represents the location of the company. Which equation represents the boundary for the region where the company delivers?•(x + 3)2 + (y– 1)2 = 49•(x + 3)2 + (y– 3)2 = 98•(x + 1)2 + (y– 3)2 = 98•(x + 3)2 + (y– 3)2 = 495. A low-watt radio station can be heard only within a certain distance from the station. On the graph below,the circular region represents that part of the city where the station can be heard, and the center of the circle represents the location of the station. Which equation represents the boundary for the region where the station can be heard?•(x– 4)2 + (y– 5)2 = 50•(x + 5)2 + (y– 5)2 = 59•(x + 5)2 + (y + 4)2 = 25•(x + 5)2 + (y– 5)2 = 256. Find the center and radius of (x– 8)2 + (y + 7)2 = 64.•(–8, 7); 8•(–7, 8); 64•(8, –7); 8•(–7, –8); 87. is tangent to circle O at B. How close to the circle is point A? (The diagram is not to scale.)• 3• 4.5• 6•7.58. Compare the quantity in Column A with the quantity in Column B.•The quantity in Column A is greater.•The quantity in Column B is greater.•The two quantities are equal.•The relationship cannot be determined from the information given.9. Solve for x.•22•710. Find the value of x.•14.6•8.1•9.4•13.411. Write the standard equation for the circle with center (14, –48) that passes through (0, 0).•(x– 14)2 + (y + 48)2 = 2500•(x + 14)2 + (y– 48)2 = 2500•(x + 14)2 + (y– 48)2 = 50•(x– 14)2 + (y + 48)2 = 5012. Find the measure of ∠BAC.•30°•150°13. Find the value of x.•79•39•99•15914. In space, which description fits the locus of points 3 cm from ?•an open cylinder of diameter 6 cm•an open cylinder of radius 3 cm and two hemispheres of diameter 6 cm each•an open cylinder of radius 3 cm and height 6 cm•an open cylinder of diameter 6 cm and two spheres of radius 3 cm each15. and are tangent to circle O and bisects ∠BPA. If m∠AOC= 68°, how much greater is m∠BCOthan m∠OAD? (The diagram is not to scale.)•22°•112°16. Write the standard equation for the circle with center (6, –8) that passes through (0, 0).•(x + 6)2 + (y– 8)2 = 0•(x– 6)2 + (y + 8)2 = 0•(x + 6)2 + (y– 8)2 = 100•(x– 6)2 + (y + 8)2 = 10017. Find the center and radius of (x + 3)2 + (y + 8)2 = 169.•(3, 8); 13•(–8, 3); 13•(–3, –8); 13•(–8, –3); 16918. , , and are all tangent to circle O. If JA = 8, AL = 13, and CK = 11, what is the perimeter ofΔJKL? (The diagram is not to scale.)•32•64•45•5319. If m = 38, what is m∠YAC?•142°•71°•109°•52°20. Write the standard equation for the circle with center (–16, 30) that passes through (0, 0).•(x + 16)2 + (y– 30)2 = 34•(x + 16)2 + (y– 30)2 = 1156•(x– 16)2 + (y + 30)2 = 1156•(x– 16)2 + (y + 30)2 = 34。
2007加拿大bc省考

English 10 – 0708 Form A
1. What does the first stanza suggest about the speaker? A. He views life as a series of insurmountable obstacles. B. He has come to appreciate the solitude of his quiet existence. C. He is relieved to have made it successfully back to the valley. D. He believes he has faced the biggest challenge he will ever face.
English 10 – 0708 Form A
Page 3
In the following poem, a mountain climber speaks about his life after climbing Mount Everest.
Page 4
Here in Katmandu
by Donald Justice
• Read the short context statement before each passage for useful information. • The numbers in the left margin next to passages tell you where to find information.
2010EuclidSolution

1.(a)Solution1Since3x=27,then3x+2=3x32=27·9=243.Solution2Since3x=27and27=33,then x=3.Therefore,3x+2=35=243.(b)Since2531359x=2731459,then x=27314592531359=2231=12.(c)The lines y=x+2and y=−12x+2both pass through the point B on the y-axis.Since the y-intercept of the line y=x+2is2,then B has coordinates(0,2). Next,wefind the x-intercepts of each of the two lines by setting y=0.If y=x+2and y=0,then x+2=0or x=−2,so A has coordinates(−2,0).If y=−12x+2and y=0,then0=−12x+2or12x=2,and so x=4.Thus,C has coordinates(4,0).Since BO and AC are perpendicular,then we can treat AC as the base of ABC and BO as its height.Note that BO=2and AC=4−(−2)=6.Therefore,the area of ABC is12×AC×BO=12×6×2=6.2.(a)Let r,g and b be the masses of the red,green and blue packages,respectively.We are told that r+g+b=60,r+g=25,and g+b=50.Subtracting the second equation from thefirst,we obtain b=60−25=35.Substituting into the third equation,we obtain g=50−b=50−35=15.Therefore,the mass of the green package is15kg.(b)Suppose that a palindrome p is the sum of the three consecutive integers a−1,a,a+1.In this case,p=(a−1)+a+(a+1)=3a,so p is a multiple of3.The largest palindromes less than200are191,181,171.Note that191and181are not divisible by3,but171is divisible by3.One way to check these without using a calculator is to use the test for divisibility by3:A positive integer is divisible by3if and only if the sum of its digits is divisibleby3.Therefore,191and181cannot be the sum of three consecutive integers.The integer171can be written as56+57+58,so171is the largest palindrome less than 200that is the sum of three consecutive integers.(c)Solution1Since(x+1)(x−1)=8,then x2−1=8or x2=9.Thus,(x2+x)(x2−x)=x(x+1)x(x−1)=x2(x+1)(x−1)=9(8)=72.Solution2Since(x+1)(x−1)=8,then x2−1=8or x2=9,so x=±3.If x=3,then(x2+x)(x2−x)=(32+3)(32−3)=(9+3)(9−3)=12(6)=72.If x=−3,then(x2+x)(x2−x)=((−3)2+(−3))((−3)2−(−3))=(9−3)(9+3)=72.In either case,(x2+x)(x2−x)=72.3.(a)Solution1Bea spends60minutesflying from H to F,30minutes at F,45minutesflying from F to G,60minutes at G,and thenflies from G to H.Thus,her total time is 60+30+45+60=195minutes plus the length of time that it takes her to fly from G to H .Since Bea flies at a constant speed,then the ratio of the two distances equals the ratio of the corresponding times.Therefore,HF GF =60minutes 45minutes =43.Since F GH is right-angled at F ,then F GH must be similar to a 3-4-5triangle,and so HG GF =53.In particular,this means that the ratio of the times flying H to G and F to G is also 53.Thus,it takes her 5×45=75minutes to fly from G to H .In conclusion,Bea is away from her hive for 195+75=270minutes.Solution 2Bea spends 60minutes flying from H to F ,30minutes at F ,45minutes flying from F to G ,60minutes at G ,and then flies from G to H .Thus,her total time is 60+30+45+60=195minutes plus the length of time that it takes her to fly from G to H .Since Bea flies at a constant speed,then the ratio of the two distances equals the ratio of the corresponding times.Therefore,we can use the Pythagorean Theorem on the times to obtainTime G to H = (Time H to F )2+(Time F to G )2=√602+452=√5625=75minsince the time is positive.In conclusion,Bea is away from her hive for 195+75=270minutes.(b)Solution 1Since ∠OP B =90◦,then OP and P B are perpendicular,so the product of their slopes is −1.The slope of OP is 4−0p −0=4p and the slope of P B is 4−0p −10=4p −10.Therefore,we need 4p ·4p −10=−116=−p (p −10)p 2−10p +16=0(p −2)(p −8)=0and so p =2or p =8.Since each these steps is reversible,then OP B is right-angled precisely when p =2and p =8.Solution 2Since OP B is right-angled at P ,then OP 2+P B 2=OB 2by the Pythagorean Theorem.Note that OB =10since O has coordinates (0,0)and B has coordinates (10,0).Also,OP 2=(p −0)2+(4−0)2=p 2+16and P B 2=(10−p )2+(4−0)2=p 2−20p +116.Therefore,(p 2+16)+(p 2−20p +116)=1022p 2−20p +32=0p 2−10p +16=0and so(p−2)(p−8)=0,or p=2or p=8.Since each these steps is reversible,then OP B is right-angled precisely when p=2and p=8.4.(a)Suppose that Thurka bought x goats and y helicopters.Then19x+17y=201.Since x and y are non-negative integers,then19x≤201so x≤10.If x=10,then17y=201−19x=11,which does not have an integer solution because11 is not divisible by17.If x=9,then17y=201−19x=30,which does not have an integer solution.If x=8,then17y=201−19x=49,which does not have an integer solution.If x=7,then17y=201−19x=68,so y=4.Therefore,19(7)+17(4)=201,and so Thurka buys7goats and4helicopters.(We can check that x=0,1,2,3,4,5,6do not give values of y that work.)(b)Solution1Manipulating algebraically,(x+8)4=(2x+16)2(x+8)4−22(x+8)2=0(x+8)2((x+8)2−22)=0(x+8)2((x+8)+2)((x+8)−2)=0(x+8)2(x+10)(x+6)=0Therefore,x=−8or x=−10or x=−6.Solution2Manipulating algebraically,(x+8)4=(2x+16)2(x+8)4−22(x+8)2=0(x+8)2((x+8)2−22)=0(x+8)2(x2+16x+64−4)=0(x+8)2(x2+16x+60)=0(x+8)2(x+10)(x+6)=0Therefore,x=−8or x=−10or x=−6.Solution3Since(x+8)4=(2x+16)2,then(x+8)2=2x+16or(x+8)2=−(2x+16).From thefirst equation,x2+16x+64=2x+16or x2+14x+48=0or(x+6)(x+8)=0.From the second equation,x2+16x+64=−2x−16or x2+18x+80=0or (x+10)(x+8)=0.Therefore,x=−8or x=−10or x=−6.5.(a)Solution1We use the fact that g(x)=g(f(f−1(x))).Since f(x)=2x+1,then to determine f−1(x)we solve x=2y+1for y to get2y=x−1or y=12(x−1).Thus,f−1(x)=12(x−1).Since g(f(x))=4x2+1,theng(x)=g(f(f−1(x)))=g(f(12(x−1)))=4(12(x−1))2+1=4·14(x−1)2+1=(x−1)2+1=x2−2x+2Solution2We use the expressions for f(x)and g(f(x))to construct g(x).Since f(x)is linear and g(f(x))is quadratic,then it is likely that g(x)is also quadratic.Since f(x)=2x+1,then(f(x))2=4x2+4x+1.Since g(f(x))has no term involving x,then we subtract2f(x)(to remove the4x term) to get(f(x))2−2f(x)=(4x2+4x+1)−2(2x+1)=4x2−1 To get g(f(x))from this,we add2to get4x2+1.Therefore,g(f(x))=(f(x))2−2f(x)+2,and so an expression for g(x)is x2−2x+2.Solution3We use the expressions for f(x)and g(f(x))to construct g(x).Since f(x)is linear and g(f(x))is quadratic,then it is likely that g(x)is also quadratic.Suppose that g(x)=ax2+bx+c for some real numbers a,b,c.Theng(f(x))=g(2x+1)=a(2x+1)2+b(2x+1)+c=a(4x2+4x+1)+b(2x+1)+c=4ax2+(4a+2b)x+(a+b+c)Since we are told that g(f(x))=4x2+1,then we can compare coefficients to deduce that 4a=4and4a+2b=0and a+b+c=1.From thefirst equation,a=1.From the second equation,b=−2a=−2.From the third equation,c=1−a−b=2.Therefore,an expression for g(x)is x2−2x+2.(b)Solution1Since the sum of thefirst two terms is40and the sum of thefirst three terms is76,then the third term is76−40=36.Since the sum of thefirst three terms is76and the sum of thefirst four terms is130,then the fourth term is130−76=54.Since the third term is36and the fourth term is54,then the common ratio in the geo-metric sequence is5436=32.Therefore,thefifth term is54·3=81and the sixth term is81·3=243.Also,the second term is 36÷32=36·23=24and the first term is 24÷32=24·23=16.Thus,the first six terms of the sequence are 16,24,36,54,81,2432.Since the first term equals 24and the common ratio is 32,then the n th term in the sequence is 24 32 n −1=3n −12n −5.When n ≥6,this is a fraction whose numerator is odd and whose denominator is even,and so,when n ≥6,the n th term is not an integer.(An odd integer is never divisible by an even integer.)Therefore,there will be 5integers in the sequence.Solution 2Suppose that a is the first term and r is the common ratio between consecutive terms (so that ar is the second term,ar 2is the third term,and so on).From the given information,a +ar =40and a +ar +ar 2=76and a +ar +ar 2+ar 3=130.Subtracting the first equation from the second,we obtain ar 2=36.Subtracting the second equation from the third,we obtain ar 3=54.Since ar 3=54and ar 2=36,then r =ar 3ar 2=5436=32.Since ar 2=36and r =32,then a (32)2=36or 94a =36or a =49·36=16.Since a =16and r =3,then the first six terms of the sequence are 16,24,36,54,81,243.Since the first term equals 24and the common ratio is 32,then the n th term in the sequence is 24 32 n −1=3n −12n −5.When n ≥6,this is a fraction whose numerator is odd and whose denominator is even,and so,when n ≥6,the n th term is not an integer.(An odd integer is never divisible by an even integer.)Therefore,there will be 5integers in the sequence.6.(a)In a 30◦-60◦-90◦triangle,the ratio of the side opposite the 90◦to the side opposite the 60◦angle is 2:√3.Note that each of ABC , ACD , ADE , AEF , AF G ,and AGH is a 30◦-60◦-90◦triangle.Therefore,AH AG =AG AF =AF AE =AE AD =AD AC =AC AB =2√3.Thus,AH =2√3AG = 2√3 2AF = 2√3 3AE = 2√3 4AD = 2√3 5AC = 2√3 6AB .(In other words,to get from AB =1to the length of AH ,we multiply by the “scalingfactor”2√3six times.)Therefore,AH = 2√36=6427.(b)Solution 1Since AF D is right-angled at F ,then by the Pythagorean Theorem,AD =√AF 2+F D 2=√42+22=√20=2√5since AD >0.Let ∠F AD =β.Since ABCD is a rectangle,then ∠BAF =90◦−β.Since AF D is right-angled at F ,then ∠ADF =90◦−β.Since ABCD is a rectangle,then ∠BDC =90◦−(90◦−β)=β.BCTherefore, BF A, AF D,and DF E are all similar as each is right-angled and has either an angle ofβor an angle of90◦−β(and hence both of these angles). Therefore,ABAF=DADFand so AB=4(2√5)2=4√5.Also,F EF D=F DF Aand so F E=2(2)4=1.Since ABCD is a rectangle,then BC=AD=2√5,and DC=AB=4√5.Finally,the area of quadrilateral BCEF equals the area of DCB minus the area DF E. Thus,the required area is1(DC)(CB)−1(DF)(F E)=1(4√5)(2√5)−1(2)(1)=20−1=19Solution2Since AF D is right-angled at F,then by the Pythagorean Theorem,AD=√AF2+F D2=√42+22=√20=2√5since AD>0.Let∠F AD=β.Since ABCD is a rectangle,then∠BAF=90◦−β.Since BAF is right-angled at F, then∠ABF=β.Since AF D is right-angled at F,then∠ADF=90◦−β.Since ABCD is a rectangle,then∠BDC=90◦−(90◦−β)=β.BCLooking at AF D,we see that sinβ=F DAD=22√5=1√5,cosβ=AFAD=42√5=2√5, and tanβ=F DAF=24=12.Since AF=4and∠ABF=β,then AB=AFsinβ=41√5=4√5.Since F D=2and∠F DE=β,then F E=F D tanβ=2·12=1.Since ABCD is a rectangle,then BC=AD=2√5,and DC=AB=4√5.Finally,the area of quadrilateral EF BC equals the area of DCB minus the area DF E. Thus,the required area is12(DC)(CB)−12(DF)(F E)=12(4√5)(2√5)−12(2)(1)=20−1=197.(a)Using the facts that 9=32and 27=33,and the laws for manipulating exponents,wehave3x −1932x 2=273x −1(32)32x 2=333x −133x 2=333x −1+3x 2=33When two powers of 3are equal,their exponents must be equal sox −1+3x 2=3x 3−x 2+3=3x 2(multiplying by x 2)x 3−4x 2+3=0Since x =1satisfies the equation,then x −1is a factor of the left ing long division or synthetic division,we can factor this out to get (x −1)(x 2−3x −3)=0.Using the quadratic formula,the quadratic equation x 2−3x −3=0has rootsx =3± (−3)2−4(1)(−3)2=3±√212Therefore,the solutions to the original equation are x =1and x =3±√212.(b)To determine the points of intersection,we equate y values of the two curves and obtainlog 10(x 4)=(log 10x )3.Since log 10(a b )=b log 10a ,the equation becomes 4log 10x =(log 10x )3.We set u =log 10x and so the equation becomes 4u =u 3,or u 3−4u =0.We can factor the left side as u 3−4u =u (u 2−4)=u (u +2)(u −2).Therefore,u (u +2)(u −2)=0,and so u =0or u =−2or u =2.Therefore,log 10x =0or log 10x =−2or log 10x =2.Therefore,x =1or x =1100or x =100.Finally,we must calculate the y -coordinates of the points of intersection.Since one of theoriginal curves is y =(log 10x )3,we can calculate the corresponding values of y by using the fact that y =u 3.The corresponding values of y are y =03=0and y =(−2)3=−8and y =23=8.Therefore,the points of intersection are (1,0),(1,−8)and (100,8).8.(a)If Oi-Lam tosses 3heads,then George has no coins to toss,so cannot toss exactly 1head.If Oi-Lam tosses 2,1or 0heads,then George has at least one coin to toss,so can toss exactly 1head.Therefore,the following possibilities exist:∗Oi-Lam tosses 2heads out of 3coins and George tosses 1head out of 1coin∗Oi-Lam tosses 1head out of 3coins and George tosses 1head out of 2coins∗Oi-Lam tosses 0heads out of 3coins and George tosses 1head out of 3coinsWe calculate the various probabilities.If 3coins are tossed,there are 8equally likely possibilities:HHH,HHT,HTH,THH,TTH,THT,HTT,TTT.Each of these possibilities has probability 1 3=1.Therefore,∗the probability of tossing 0heads out of 3coins is 18∗the probability of tossing 1head out of 3coins is 38∗the probability of tossing 2heads out of 3coins is 38∗the probability of tossing 3heads out of 3coins is 18If 2coins are tossed,there are 4equally likely possibilities:HH,HT,TH,TT.Each of these possibilities has probability 12 2=14.Therefore,the probability of tossing 1head out of 2coins is 2=1.If 1coin is tossed,the probability of tossing 1head is 12.To summarize,the possibilities are∗Oi-Lam tosses 2heads out of 3coins (with probability 38)and George tosses 1head out of 1coin (with probability 12)∗Oi-Lam tosses 1head out of 3coins (with probability 38)and George tosses 1head out of 2coins (with probability 12)∗Oi-Lam tosses 0heads out of 3coins (with probability 18)and George tosses 1head out of 3coins (with probability 38)Therefore,the overall probability is 38·12+38·12+18·38=2764.(b)Suppose ∠P AR =x ◦and ∠QDR =y ◦.Since P R and P A are radii of the larger circle,then P AR is isosceles.Thus,∠P RA =∠P AR =x ◦.Since QD and QR are radii of the smaller circle,then QRD is isosceles.Thus,∠QRD =∠QDR =y ◦.In ARD ,the sum of the angles is 180◦,so x ◦+(x ◦+40◦+y ◦)+y ◦=180◦or 2x +2y =140or x +y =70.Therefore,∠CP D =x ◦+40◦+y ◦=(x +y +40)◦=110◦.9.(a)(i)Solution 1LS =cot θ−cot 2θ=cos θsin θ−cos 2θsin 2θ=sin 2θcos θ−cos 2θsin θsin θsin 2θ=sin(2θ−θ)sin θsin 2θ=sin θsin θsin 2θ=1sin 2θ=RSas required.Solution2LS=cotθ−cot2θ=cosθsinθ−cos2θsin2θ=cosθsinθ−cos2θ2sinθcosθ=2cos2θ−cos2θ2sinθcosθ=2cos2θ−(2cos2θ−1)sin2θ=1 sin2θ=RS as required.(ii)We use(i)to note that1sin8◦=cot4◦−cot8◦and1sin16◦=cot8◦−cot16◦and soon.Thus,S=1sin8◦+1sin16◦+1sin32◦+···+1sin4096◦+1sin8192◦=(cot4◦−cot8◦)+(cot8◦−cot16◦)+(cot16◦−cot32◦)+···+(cot2048◦−cot4096◦)+(cot4096◦−cot8192◦)=cot4◦−cot8192◦since the sum“telescopes”.Since the cotangent function has a period of180◦,and8100◦is a multiple of180◦, then cot8192◦=cot92◦.Therefore,S=cot4◦−cot92◦=cos4◦sin4◦−cos92◦sin92◦=cos4◦sin4◦−−sin2◦cos2◦=cos4◦2sin2◦cos2◦+sin2◦cos2◦=cos4◦+2sin22◦2sin2◦cos2◦=(1−2sin22◦)+2sin22◦sin4◦=1 sin4◦Therefore,α=4◦.(b)Solution 1We use the notation A =∠BAC ,B =∠ABC and C =∠ACB .We need to show that A <12(B +C ).Since the sum of the angles in ABC is 180◦,then B +C =180◦−A ,and so this inequality is equivalent to A <12(180◦−A )which is equivalent to 32A <90◦or A <60◦.So we need to show that A <60◦.We know that a <12(b +c ).Thus,2a <b +c and so 4a 2<b 2+c 2+2bc because all quantities are positive.Using the cosine law in ABC ,we obtain a 2=b 2+c 2−2bc cos A .Therefore,4a 2<b 2+c 2+2bc 4(b 2+c 2−2bc cos A )<b 2+c 2+2bc 4b 2+4c 2−8bc cos A<b 2+c 2+2bc 4b 2+4c 2−8bc cos A<b 2+c 2+2bc +3(b −c )2(since (b −c )2≥0)4b 2+4c 2−8bc cos A<b 2+c 2+2bc +3b 2−6bc +3c 24b 2+4c 2−8bc cos A<4b 2+4c 2−4bc −8bc cos A<−4bc cos A >12(since 8bc >0)Since 2a <b +c ,then a cannot be the longest side of ABC (that is,we cannot have a ≥b and a ≥c ),so A must be an acute angle.Therefore,cos A >12implies A <60◦,as required.Solution 2We use the notation A =∠BAC ,B =∠ABC and C =∠ACB .We need to show that A <12(B +C ).Since the sum of the angles in ABC is 180◦,then B +C =180◦−A ,and so this inequality is equivalent to A <12(180◦−A )which is equivalent to 32A <90◦or A <60◦.So we need to show that A <60◦.We know that a <12(b +c )which implies 2a <b +c .Using the sine law in ABC ,we obtain a sin A =b sin B =c sin C ,which gives b =a sin B sin A and c =a sin C sin A .Therefore,we obtain equivalent inequalities2a <b +c2a <a sin B sin A +a sin C sin A2a sin A <a sin B +a sin C(since sin A >0for 0◦<A <180◦)2sin A <sin B +sin C since a >0.Next,we use the trigonometric formula sin B +sin C =2sin B +C 2 cos B −C 2 .Since cos θ≤1for any θ,then sin B +sin C ≤2sin B +C 2 ·1=2sin B +C 2.Therefore,2sin A<sin B+sin C≤2sinB+C22sin A<2sinB+C22sin A<2sin180◦−A24sin(12A)cos(12A)<2sin(90◦−12A)2sin(12A)cos(12A)<cos(12A)Since0◦<A<180◦,then cos(1A)>0,so sin(1A)<1.Since2a<b+c,then a cannot be the longest side of ABC,so A must be an acute angle.Therefore,1A<30◦or A<60◦,as required.10.Denote the side lengths of a triangle by a,b and c,with0<a≤b≤c.In order for these lengths to form a triangle,we need c<a+b and b<a+c and a<b+c.Since0<a≤b≤c,then b<a+c and a<b+c follow automatically,so only c<a+b ever needs to be checked.Instead of directly considering triangles and sets of triangle,we can consider triples(a,b,c)and sets of triples(a,b,c)with the appropriate conditions.For each positive integer k≥3,we use the notation S k to denote the set of triples of positive integers(a,b,c)with0<a≤b≤c and c<a+b and a+b+c=k.In this case,c<a+b and a+b+c=k,so c+c<a+b+c=k,so2c<k or c<12k.Also,if0<a≤b≤c and a+b+c=k,then k=a+b+c≤c+c+c,so3c≥k or c≥13k.(a)Consider T(10),which is the number of elements in S10.We want tofind all possible triples(a,b,c)of integers with0<a≤b≤c and c<a+b and a+b+c=10.We need c<102=5and c≥103.Thus,c=4.Therefore,we need0<a≤b≤4and a+b=6.There are two possibilities:(a,b,c)=(2,4,4)or(a,b,c)=(3,3,4).Therefore,T(10)=2.Consider T(11).We want tofind all possible triples(a,b,c)of integers with0<a≤b≤c and c<a+b and a+b+c=11.We need c<112and c≥113.Thus,c=4or c=5.If c=4,we need0<a≤b≤4and a+b=7.There is only one possibility:(a,b,c)=(3,4,4).If c=5,we need0<a≤b≤5and a+b=6.There are three possibilities:(a,b,c)=(1,5,5)or(a,b,c)=(2,4,5)or(a,b,c)=(3,3,5). Therefore,T(11)=4.Consider T(12).We want tofind all possible triples(a,b,c)of integers with0<a≤b≤c and c<a+b and a+b+c=12.We need c<122and c≥123.Thus,c=4or c=5.If c=4,we need0<a≤b≤4and a+b=8. There is only one possibility:(a,b,c)=(4,4,4).If c=5,we need0<a≤b≤5and a+b=7.There are two possibilities:(a,b,c)=(2,5,5)or(a,b,c)=(3,4,5).Therefore,T(12)=3.(b)We show that T(2m)=T(2m−3)by creating a one-to-one correspondence between thetriples in S2m and the triples S2m−3.Note that S2m is the set of triples(a,b,c)of positive integers with0<a≤b≤c,with c<a+b,and with a+b+c=2m.Also,S2m−3is the set of triples(A,B,C)of positive integers with0<A≤B≤C,with C<A+B,and with A+B+C=2m−3.Consider a triple(a,b,c)in S2m and a corresponding triple(a−1,b−1,c−1).We show that(a−1,b−1,c−1)is in S2m−3:∗Since(a,b,c)is in S2m,then c<12(2m)=m.This means that b≤c≤m−1,soa=2m−b−c≥2.Therefore,a−1,b−1and c−1are positive integers since a,b and c are positive integers with2≤a≤b≤c.∗Since2≤a≤b≤c,then1≤a−1≤b−1≤c−1,so0<a−1≤b−1≤c−1.∗Since a+b+c=2m,then c=2m−(a+b)so a+b and c have the same parity.Since c<a+b,then c≤a+b−2.(In other words,it cannot be the case that c=a+b−1.)Therefore,c−1≤(a−1)+(b−1)−1;that is,c−1<(a−1)+(b−1).∗Since a+b+c=2m,then(a−1)+(b−1)+(c−1)=2m−3.Therefore,(a−1,b−1,c−1)is in S2m−3,since it satisfies all of the conditions of S2m−3.Note as well that two different triples in S2m correspond to two different triples in S2m−3.Thus,every triple in S2m corresponds to a different triple in S2m−3.Thus,T(2m)≤T(2m−3).Consider a triple(A,B,C)in S2m−3and a corresponding triple(A+1,B+1,C+1).We show that(A+1,B+1,C+1)is in S2m:∗Since(A,B,C)is in S2m−3,then A,B and C are positive integers,so A+1,B+1 and C+1are positive integers.∗Since0<A≤B≤C,then1<A+1≤B+1≤C+1,so0<A+1≤B+1≤C+1.∗Since C<A+B,then C+1<(A+1)+(B+1)−1so C+1<(A+1)+(B+1).∗Since A+B+C=2m−3,then(A+1)+(B+1)+(C+1)=2m.Therefore,(A+1,B+1,C+1)is in S2m.Note again that two different triples in S2m−3correspond to two different triples in S2m.Thus,every triple in S2m−3corresponds to a different triple in S2m.Therefore,T(2m−3)≤T(2m).Since T(2m)≤T(2m−3)and T(2m−3)≤T(2m),then T(2m)=T(2m−3).(c)We will use two important facts:(F1)T(2m)=T(2m−3)for every positive integer m≥3,and(F2)T(k)≤T(k+2)for every positive integer k≥3We proved(F1)in(b).Next,we prove(F2):Consider a triple(a,b,c)in S k and a corresponding triple(a,b+1,c+1).Weshow that the triple(a,b+1,c+1)is in S k+2:∗Since a,b and c are positive integers,then a,b+1and c+1are positiveintegers.∗Since0<a≤b≤c,then0<a≤b+1≤c+1.∗Since c<a+b,then c+1<a+(b+1).∗Since a+b+c=k,then a+(b+1)+(c+1)=k+2.Therefore,(a,b+1,c+1)is in S k+2.Note that,using this correspondence, different triples in S k correspond different triples in S k+2.Thus,every triple in S k corresponds to a different triple in S k+2.This proves that T(k)≤T(k+2). Suppose that n=N is the smallest positive integer for which T(n)>2010.Then N must be odd:If N was even,then by(F1),T(N−3)=T(N)>2010and so n=N−3would be an integer smaller than N with T(n)>2010.This contradicts the fact that n=N is the smallest such integer.Therefore,we want tofind the smallest odd positive integer N for which T(N)>2010. Next,we note that if we canfind an odd positive integer n such that T(n)>2010≥T(n−2),then we will have found the desired value of n:This is because n and n−2are both odd,and by property(F2),any smaller odd positive integer k will give T(k)≤T(n−2)≤2010and any larger odd positive integer m will give T(m)≥T(n)>2010.We show that N=309is the desired value of N by showing that T(309)>2010and T(307)≤2010.Calculation of T(309)We know that3093≤c<3092,so103≤c≤154.For each admissible value of c,we need to count the number of pairs of positive integers (a,b)with a≤b≤c and a+b=309−c.For example,if c=154,then we need a≤b≤154and a+b=155.This gives pairs(1,154),(2,153),...,(76,79),(77,78),of which there are77.Also,if c=153,then we need a≤b≤153and a+b=156.This gives pairs(3,153),...,(77,79),(78,78),of which there are76.In general,if c is even,then the minimum possible value of a occurs when b is as large as possible–that is,when b=c,so a≥309−2c.Also,the largest possible value of a occurs when a and b are as close to equal as possible. Since c is even,then309−c is odd,so a and b cannot be equal,but they can differ by1.In this case,a=154−12c and b=155−12c.Therefore,if c is even,there are(154−1c)−(309−2c)+1=3c−154possible pairs(a,b)and so32c−154possible triples.In general,if c is odd,then the minimum possible value of a occurs when b is as large as possible–that is,when b=c,so a≥309−2c.Also,the largest possible value of a occurs when a and b are as close to equal as possible.Since c is odd,then309−c is even,so a and b can be equal.In this case,a=12(309−c).Therefore,if c is odd,there are1(309−c)−(309−2c)+1=3c−307possible pairs(a,b)and so32c−3072possible triples.The possible even values of c are104,106,...,152,154(there are26such values)and the possible odd values of c are103,105,...,151,153(there are26such values).Therefore,T(309)= 32(104)−154+32(106)−154+···+32(154)−154+ 32(103)−3072+32(105)−3072+···+32(153)−3072=32(104+106+···+154)−26·154+32(103+105+···+153)−26·3072=32(103+104+105+106+···+153+154)−26·154−26·3072=32·12(103+154)(52)−26·154−26·3072=32(26)(257)−26·154−26·3072=2028Therefore,T(309)>2010,as required.Calculation of T(307)We know that307≤c<307,so103≤c≤153.For each admissible value of c,we need to count the number of pairs of positive integers (a,b)with a≤b≤c and a+b=307−c.This can be done in a similar way to the calculation of T(309)above.If n is even,there are32c−153possible triples.If n is odd,there are32c−3052possible triples.The possible even values of c are104,106,...,150,152(there are25such values)and the possible odd values of c are103,105,...,151,153(there are26such values).Therefore,T(307)= 32(104)−153+32(106)−153+···+32(152)−153+ 32(103)−3052+32(105)−3052+···+32(153)−3052=32(104+106+···+152)−25·153+32(103+105+···+153)−26·3052=32(103+104+105+106+···+152+153)−25·153−26·3052=3·1(103+153)(51)−25·153−26·305=32(51)(128)−25·153−26·3052=2002Therefore,T(307)<2010,as required.Therefore,the smallest positive integer n such that T(n)>2010is n=309.As afinal note,we discuss briefly how one could guess that the answer was near N=309.Consider the values of T(n)for small odd positive integers n.In(a),by considering the possible values of c from smallest(roughly13n)to largest(roughly12n),we saw that T(11)=1+3=4.If we continue to calculate T(n)for a few more small odd values of n we will see that:T(13)=2+3=5T(15)=1+2+4=7T(17)=1+3+4=8T(19)=2+3+5=10T(21)=1+2+4+5=12T(23)=1+3+4+6=14The pattern that seems to emerge is that for n odd,T(n)is roughly equal to thesum of the integers from1to14n,with one out of every three integers removed.Thus,T(n)is roughly equal to23of the sum of the integers from1to14n.Therefore,T(n)≈23·12(14n)(14n+1)≈23·12(14n)2≈148n2.It makes sense to look for an odd positive integer n with T(n)≈2010.Thus,we are looking for a value of n that roughly satisfies148n2≈2010orn2≈96480or n≈310.Since n is odd,then it makes sense to consider n=309,as in the solution above.。
加拿大数学10年级练习第二部分

1. Solve – + = 4. Check your solution.•5, –2• 5•6, –1• 62. Solve = . Check your solution.•–•8• 4•no solution3. Divide ÷ .••••4. Solve –= 1. Check your solution.•0•–7•–7, 0•6, –75. The graph of the function y = is on the left. The graph on the right is the same graph translated right threeunits and up three units. What is the equation of the graph on the right?•y = + 3•y = – 3•y = + 3•y = – 36. Divide (11x2 + 2x3 + 14 + 17x) ÷ (2x + 5).•x2 + 3x + 2 +•x2 + 3x + 1 +•x2– 3x– 1 +•x2– 3x– 2 +7. If no digit appears more than once, how many 2-digit numbers can be formed from the digits 2, 3, 5, 6?• 6• 4•12•658. Divide ÷ (x– 2).•••9. Divide (–3x3 + 4x–5) ÷ (x + 3).•–3x2 + 9x– 23 +•–3x2 + 13x + 39 –•–3x2 + 9x + 31 –•–3x2 + 13x– 44 +10. Divide ÷ .••••11. Multiply • .••••12. Simplify .•••13. Divide (6x2– 5x+ 2) ÷ (3x + 2).•2x + 3•2x– 3 –•2x + 3 –•2x– 3 +14. Simplify .•x2– 9••–x– 5•x + 515. Jamestown Builders has a development of new homes. There are six different floor plans, five exterior colors,and an option of either a two-car or a three-car garage. How many choices are there for one home?•30•90•60•5216. Divide ÷ (x + 6).••••17. Simplify .•••18. The rate of discount R, can be determined using the formula R = 1 –, where P is the regular price ofan item and D is the discount, or amount saved off the regular price. Find the rate of discount on a sweater witha regular price of $28.99 that is on sale for $10.99.•37.9%•$10.99•$18•62.1%19. Fifteen students volunteer for a committee. How many different eight-person committees can be chosen?•6435 committees•40,320 committees•5040 committees•259,459,200 committees20. Divide (2x2 + 4x3– 9 – 10x) ÷ (2x + 3).•2x2– 2x– 1 –•2x2 + 2x + 2 –•2x2 + 2x + 1 –•2x2– 2x– 2 –1. Identify the graph of 2x– 5y = –10.••••2. Write an equation for the translation of y = |x| left 0.3 units.•y = |x| – 0.3•y = |x| + 0.3•y = |x– 0.3|•y = |x + 0.3|3. Which is the graph of y = |x + 4| – 2?••••4. The rate of change is constant in the graph. Find the rate of change. Explain what the rate of change means forthe situation.•–200, value drops $200 every year•–, value drops $200 every 3 years•–20, value drops $20 every year•–60, value drops $60 every year5. Identify the graph of y = –|x– 5| by translating y = –|x|.••••6. Identify the graph of –10x + 5y = –50.••••7. A line passes through (–7, 1) and (4, 4). Identify the point-slope and slope-intercept form of an equation ofthe line.•point-slope: y– 1 = (x + 7); slope-intercept: y = x +•point-slope: y + 7 = (x– 1); slope-intercept: y = x–•point-slope: y– 1 = (x + 7); slope-intercept: y = x +•point-slope: y + 7 = (x– 1); slope-intercept: y = x–8. As water is poured into a tank, the volume was measured every minute. It produced the graph below. What was thevolume at three minutes?• 3.0• 6.0• 4.0•7.99. The table shows the time spent researching the stock market each week and the average weekly percent gain foran investor over one year. Use a trend line to predict the average weekly percent gain from researching the stock market for 20 hours per week.•about 10%•about 20%•about 0.5%•about 8%10. Write an equation for the translation of y = |x| down 0.75 units.•y = |x+ 0.75|•y = |x| + 0.75•y = |x| – 0.75•y = |x– 0.75|11. Identify the graph of y = |x| + 3.5 by translating y = |x|.••••12. Which is the graph of y = |x + 5| + 3?••••13. Graph the data for the times Shauna spent learning music for the violin and her scores at the annual solocompetitions. Find an equation of the trend line of the data.•y– 31 = 2.5(x– 10)•x– 31 = 2.5(y– 10)•y– 10 = 2.5(x– 21)•y + 31 = 2.5(x + 10)14. Find the slope of the line.• 6•–6••–15. Find the slope of the line.•–1• 5•–5•undefined16. Write the slope-intercept form of the equation for the line.•y = –x + 7•y = x– 7•y = –x– 7•y = x + 717. Find the slope of a line perpendicular to the graph of 5x + y = 7.•–5••–• 518. Describe the relationship between the average June temperatures and the latitude positions of cities on thescatter plot below.•The temperatures are about the same at all latitudes.•The lower the latitude, the lower the temperature.•The higher the latitude, the lower the temperature.•The higher the latitude, the higher the temperature.19. Find the slope of the line.•–•–3•0•undefined20. The rate of change is constant in the graph. Find the rate of change. Explain what the rate of change meansfor the situation.•–20, value drops $20 every year•–60, value drops $60 every year•–, value drops $200 every 3 years•–200, value drops $200 every year1. You decide to market your own custom computer software. You must invest $4305 on computer hardware, and spend$2.75 to buy and package each disk. If each program sells for $13.00, how many copies must you sell to break even?•421 copies•274 copies•273 copies•420 copies2. Solve by elimination.3x– 4y = 9–3x + 2y = 9•(–27, –9)•(–9, –9)•(–3, –6)•(3, 9)3. You decide to market your own custom computer software. You must invest $3255 on computer hardware, and spend$2.90 to buy and package each disk. If each program sells for $13.75, how many copies must you sell to break even?•195 copies•301 copies•196 copies•300 copies4. What is the graph of y > –6x + 3?••••5. Which ordered pair is a solution of the system?7x + 2y = –39–8x + 3y = –3•(–3, –9)•(–7, –11)•(–4, –7)•(–1, –10)6. Solve by elimination.4x–y = 43x + y = 10•(0, –4)•(12, 4)•(2, 4)•(4, 12)7. The length of a rectangle is 3 centimeters more than 3 times the width. If the perimeter of the rectangle is46 centimeters, what are the dimensions of the rectangle?•length = 5 cm; width = 18 cm•length = 13 cm; width = 5 cm•length = 13 cm; width = 8 cm•length = 18 cm; width = 5 cm8. Which point is a solution of y≥ 4x– 5?•(1, 1)•(2, 1)•(3, 0)•(3, 4)9. Which system has infinitely many solutions?•x + y = 3x + y = 4•2x + y = 54x + 2y = 10•2x + y = 74x– 2y = 14•3x–y = 2x– 3y = –210. Solve the system using substitution.f(x) = x– 4f(x) = x– 6•(3, –3)•(3, –4)•(6, –2)•(4, 6)11. A motorboat can go 16 miles downstream on a river in 20 minutes. It takes 30 minutes for this boat to go backupstream the same 16 miles. Find the speed of the boat in still water.•40 mi/h•32 mi/h•8 mi/h•48 mi/h12. Which system has no solution?•4x– 2y = 1y = 2x– 7•y = 2x + 2x– 2y = 1•y = –x + 1x–y = 1•3x–y = 3y = –3x + 313. A motorboat can go 8 miles downstream on a river in 20 minutes. It takes 30 minutes for this boat to go backupstream the same 8 miles. Find the speed of the current.•20 mi/h•16 mi/h•24 mi/h• 4 mi/h14. Find the solution of the system.3y = –x + 2y = –x + 9•(3, 6)•(20, –4)•(10, –1)•(–1, 8)15. Mrs. Huang operates a soybean farm outside Grinnell, Iowa. To keep her operating costs down, she buys many productsin bulk and transfers them to smaller containers for use on the farm. Often the bulk products are not the correct concentration and need to be custom mixed before Mrs. Huang can use them. One day she wants to apply herbicide toa large field. A solution of 67% herbicide is to be mixed with a solution of 46% herbicide to form 42 liters ofa 55% solution. How much of the 67% solution must she use?•34 L•23 L•18 L•35 L16. What is the graph of y≤ 2x– 2?••••17. Write x– 2y < –2 in slope-intercept form. Then graph the inequality.•y > x + 1•y < x + 1•y < –x + 1•y > –x + 118. Which graphing calculator screen shows the solution of the system?y = –x + 3y = 6x– 5••••19. Identify the graph of y≤ 3x– 3.••••20. Mike and Kim invest $10,000 in equipment to print yearbooks for schools. Each yearbook costs $5.75 to printand sells for $25. How many yearbooks must they sell before their business breaks even?•500 yearbooks•520 yearbooks•400 yearbooks•1,740 yearbooks1. This graph represents a translation of the graph of y = |x|. What is the equation of this graph?•y = |x− 2|•y = |x| − 2•y= |x + 2|•y = |x| + 22. Find an equation for the linear model of the situation below and use it to make a prediction. A train is travelingnorth at a constant rate. At 3:00 P.M. it is 55 miles north of a city. At 4:15 P.M. it is 80 miles north of the city.If d represents the distance in miles, and t represents the time in hours, how many miles north of the city will the train be at 5:45 P.M.?•d = 64t + 55; d = 231 miles•d = 80t + 55; d = 255 miles•d = 64t + 55; d = 96 miles•d = 100t + 55; d = 285 miles3. What are the domain and range of this function?•Domain: x > 0; Range: y > 0•Domain: x≥ 0; Range: y≥ 0•Domain: all real numbers; Range: all real numbers•Domain: positive integers; Range: positive integers4. What is the graph of y > |x− 3|?••••5. An electronics store makes a profit of $55 for every standard CD player sold and $77 for every portable CD playersold. The manager's goal is to make at least $385 a day on sales of both standard and portable CD players. If s represents the number of standard CD players sold, and p represents the number of portable CD players sold, which inequality and graph represent the manager's daily goal?•55s + 77p≤ 385•77s + 55p≥ 385•55s + 77p≥ 385•77s + 55p≤ 3856. What is an equation of the inequality?•y≤ |x− 1| − 2•y≥ |x + 1| − 2•y≥ |x− 1| − 27. What is the graph of y≥ |x + 1| − 1?••••8. What is the graph of y = −|3x|?•••9. What is the graph of y = |−x− 4|?•••10. What is the equation of y = |x| translated 1 unit up and 6 units to the left?•y = |x− 6| + 1•y = |x− 1| + 6•y = |x + 1| + 6•y = |x + 6| + 111. What is the graph of y = |x| − |2x|?••••not here12. The scatter plot below shows the weights in ounces of several kittens at various ages. What is the best equationof the trend line through these points?•y = 10x•y = 4x•y = x + 10•y = 4x + 1013. What is the standard form of the equation of the line whose slope is −2 and which contains the point (3, −5)?•2x + y = 1•2x + y = −8•2x + y = 11•2x + y = −714. Find f(−5) if f(x) = −8x− 1.•39•−41•−39•1215. The points in the table have been graphed in the scatter plot below. What is the best equation for the trendline, and the value of y when x is 16?•y = 1.25x + 1; y = 21•y = −1.25x + 1; y = 19•y = 2x + 1; y = 33•y = 0.8x + 1; y = 11.816. A linear model for the situation below passes through the origin.A manufacturer can produce 4200 gears in 8 hours. How many gears can be made in 1 hour?•525•262.5•1050•60017. What is an inequality for this graph?•y− 3x > −5•y− 3x≥ −5•y− 3x≤ −5•y− 3x < −518. Which set of points represents the graph of the function below?(−3, 2), (−1, −1), (2, 2), (4, 0)••••19. What is the graph of the line whose slope is and whose y-intercept is 4?••••20. The graph below models the burning of a candle. At time t = 0, the candle was 8 inches tall. If the candle burnsat a rate of 2 inches per hour, what equation models the height h of the candle after burning for t hours?•h = 2t + 8•h = 8t + 2•h = 8t− 2•h = −2t + 81. Which system of equations is the result of using the elimination method to solve the system ,and what is the solution?•; (1, 12)•; (11, 13)•; (2, )•; (13, 4)2. Which point lies in the plane represented by the equation 2x + 7y + 3z = 25?•(−6, −4, 5)•(3, −3, 3)•(−3, 3, −2)•(6, 1, −3)3. The equation P= 5x+ 3y represents the objective function for this linear programming model. What is the vertexof the feasible region shown below that results in the maximum value of P?•(12, 14)•(12, 2)•(14, 4)•(8, 12)4. Use elimination to find the solution of the system .•(−1, , 3)•(−1, 3, 2)•(1, 3, 0)•(−1, 2, 4)5. Find the values of x and y that maximize the objective function for this graph.Maximum for P = 3x + 2y•(0, 9)•(0, 0)•(5, 0)•(1, 8)6. Which system is inconsistent?••••7. A point (x, y, z) can be used to represent the location of a geographical point in space, where x representsthe latitude in degrees, y represents the longitude in degrees, and z represents the elevation in feet above or below sea level.Jerimoth Hill is the highest point of elevation in Rhode Island. It is 812 feet above sea level and lies at about 42° north latitude and 72° west longitude. What ordered triple represents the location of Jerimoth Hill?•(42°, −72°, −812)•(42°, −72°, 812)•(−72°, 42°, 812)•(−72°, 42°, −812)8. What is the graph of −3x + 5y +10z = 15?••••9. What steps would you use to plot (−3, −4, 0) in a three-dimensional coordinate system?•From the origin, move back 3 units and down 4 units.•From the origin, move back 3 units and left 4 units.•From the origin, move down 3 units and left 4 units.•From the origin, move back 3 units and up 4 units.10. Identify the graph of the system and its solution.•(3, 0)•(−3, 0)•infinite number of solutions•no solution11. What is the solution of this system?•(, )•(−2, −3)•(−14, −23)•(−3, −2)12. A small fish market sells only tuna and salmon. A tuna costs the fish market $0.75 per pound to buy and $2.53per pound to clean and package. A salmon costs the fish market $3.00 per pound to buy and $2.75 per pound to clean and package. The fish market makes $2.50 per pound profit for each tuna it sells and $2.80 per pound profit for each salmon it sells. The fish market owner can spend only $159.00 per day to buy fish and $197.34 per day to clean and package the fish.The graph below represents the feasible region for this linear programming model. What are the coordinates of the vertices of the feasible region, and what are the vales of t and s that maximize the objective function?; P = 2.50t + 2.80s•(0, 0), (0, 53), (71.76, 0), (46, 28); t = 46 and s = 28.•(0, 0), (53, 0), (0, 71.76), (28, 46); t = 28 and s = 46.•(0, 0), (0, 53), (71.76, 0), (28, 46); t = 28 and s = 46.•(0, 0), (0, 53), (71.76, 0), (46, 28); t = 0 and s = 53.13. Identify the graph of the system and its solution.•(−5, −4)•(, −)•(, −)•(−1, 8)14. Identify the graph that shows the solution to the system of inequalities.••••15. What is the maximum for the objective function for this graph?Maximum for P = 5x + 2y•90•80•250•56016. How could you solve this system using substitution?•Substitute for r in the equation t = 2r + 3.•Substitute for t in the equation t = 2r + 3.•Substitute 2r + 3 for t in the equation 5r− 4t = 6.•Substitute 2r + 3 for r in the equation 5r− 4t = 6.17. How could you solve this system by substitution?•Substitute 6 for c in the equation 3c + 5d = 2.•Substitute 6 for d into the equation 3c + 5d = 2.•Substitute for d into the equation d = 6.•Substitute 3(3c + 5d) for 6 in the equation d = 6.18. Identify the graph that shows the solution to the system of inequalities.••••19. Identify the graph that shows the solution to the system of inequalities.••••20. Locate the point (4, −3, 3) in a three-dimensional coordinate system.••••none of these。
加拿大数学10年级练习第一部分几何
1. ABCD is an isosceles trapezoid, and ≅. Which statement is NOT true?•ΔABY≅ΔBAX•≅•≅•∠DXB≅∠DYB2. It appears from the name of the HL Theorem that you actually need to know only two parts of a triangle in orderto prove two triangles congruent. Is this the case?•Yes, you only need to know the hypotenuse and a leg of a triangle.•No, you actually need to know two sides and an angle, because the triangle must be a right triangle.•No, you actually need to know three sides of the triangle.•No, you actually need to know two angles and a side.3. Complete the proof.Given: ≅, ∠1 ≅∠2, and ≅.Prove: ΔCEF≅ΔAEF.∠BEC≅∠DEA by vertical angles. ΔBEC≅ΔDEA by (a)_____. Then by CPCTC, ≅. ≅by the Reflexive Property. So ΔCEF≅ΔAEF by (b)_____.• a. SAS; b. SAS• a. AAS; b. SSS• a. ASA; b. SSS• a. AAS; b. HL4. Complete the proof.Given: ≅, ∠1 ≅∠2Prove: ΔBEA≅ΔDEC≅and ∠1 ≅∠2, so ΔBCA≅ΔDAC by SAS. Then, since (a)_____, ≅, ≅. ∠BEA≅∠DEC by (b)_____, so ΔBEA≅ΔDEC by (c)_____.• a. CPCTC; b. vertical angles; c. SAS• a. CPCTC; b. vertical angles; c. AAS• a. CPCTC; b. vertical angles; c. SSS• a. SAS; b. vertical angles; c. SSS5. What additional information can be used to prove the triangles congruent by the HL Theorem?•m∠BCE = 90•AB > AC•≅•≅6. Suppose ΔCED≅ΔDBC. If m∠EDC = 63 and m∠DBC = 82, what is m∠DCE?•63•82•145•357. If ∠A≅∠D and ∠C≅∠F, which statement would NOT prove that ΔABC≅ΔDEF?••∠B≅∠E•≅•none of these8. Determine what information you would need to know in order to use the SSS Congruence Postulate to show that thetriangles are congruent.•∠BAD≅∠CDB•≅•∠ADB≅∠CBD•≅9. Suppose ΔBCA≅ΔECD. Which statement is NOT necessarily true?•≅•∠A≅∠D•≅•∠BCA≅∠DCE10. In which triangles could you efficiently prove Δ1 ≅Δ2 using the HL Theorem?•II only•III only•II and III•I only11. Complete the proof.Given: ≅, ∠1 ≅∠2, and ≅.Prove: ΔCEF≅ΔAEF.∠BEC≅∠DEA with vetical angles. ΔBEC≅ΔDEA by (a)_____. Then by (b)_____, ≅. ≅by the Reflexive Property. So ΔCEF≅ΔAEF by (c)_____.• a. AAS; b. CPCTC; c. SSS• a. SAS; b. CPCTC; c. SSS• a. AAS; b. CPCTC; c. SAS• a. SSS; b. CPCTC; c. ASA12. In the paper airplane, ABCD≅EFGH, m∠B = m∠BCD = 90, and m∠BAD = 140. Find m∠GHE.•130•90•40•14013. Find the value of x.•x = –2•x = 9•x = 21•none of these14. Explain how you can use SSS, SAS, ASA, or AAS with CPCTC to prove that ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Symmetric Property, ≅. By SAS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By ASA, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By SAS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.•≅and ∠ACB≅∠ACD. By the Reflexive Property, ≅. By SSS, ΔABC≅ΔADC, so by CPCTC ∠D≅∠B.15. Complete the proof.Given: bisects ∠URS and bisects ∠UTS.Prove: ΔURT≅ΔSRT.•Reflexive property•definition of angle bisector•HL Theorem•CPCTC16. Complete the proof.Given: ∠RSQ≅∠TSQ, ∠RQS≅∠TQS.Prove: ≅.∠RSQ≅∠TSQ is given, as is ∠RQS≅∠TQS. By the Reflexive Property, ≅.ΔSRQ≅ΔSTQ by (a)_____, so ≅by (b)_____.• a. ASA; b. CPCTC• a. HL; b. CPCTC• a. SSS; b. CPCTC• a. SAS; b. CPCTC17. Determine which triangles are congruent by AAS using the information in the diagram below.•ΔABF≅ΔEDF•ΔADC≅ΔEBC•ΔABE≅ΔEDA•ΔABE≅ΔCBE18. Complete the proof.Given: bisects ∠EBC and bisects ∠ECC.Prove:ΔEBD≅ΔCBD.•Same-Side Interior Angles Theorem•given•SSS postulate•Triangle Inequality Theorem19. ΔABD≅ΔCBD. Name the theorem or postulate that justifies the congruence.•SAS•AAS•ASA•none of these1. Determine whether each quadrilateral can be a parallelogram. If not, write impossible.a. Two adjacent angles are right angles, but the quadrilateral is not a rectangle.b. All of the angles are congruent.• a. impossible; b. parallelogram• a. parallelogram; b. parallelogram• a. parallelogram; b. impossible• a. impossible; b. impossible2. Which statement is true?•All rectangles are squares.•All quadrilaterals are squares.•All quadrilaterals are parallelograms.•All parallelograms are quadrilaterals.3. Which statement can be used to determine whether quadrilateral XYZW must be a parallelogram?•≅and ≅•≅and ≅•≅and ≅•XW = WZ and XY = YZ4. Choose the best name for the parallelogram and find the measures of the numbered angles.•Square; all numbered angles are equal to 45°.•Rhombus; all numbered angles are equal to 115°.•Rhombus; all numbered angles are equal to 25°.•Square; all numbered angles are equal to 50°.5. Given: quadrilateral ABCD with A(–2, 3), B(2, –4), C(9, 0), D(5, 7). Then ABCD is a rectangle because•the slopes of the sides in pairs are negative reciprocals.•the product of the slopes of the diagonals is –1.•the figure has four vertices.•opposite sides have the same slope.6. Given the parallelogram below, find coordinates for P, without using any new variables.•(a–c, b)•(a + c, b)•(c, b)•(c, a)7. Find the values of the variables for the rectangle. Then find the lengths of the sides.•x = 7, y = 5; side lengths: 70, 45•x = 5, y = 7; side lengths: 33, 94•x = 5, y = 7; side lengths: 50, 63•x = 7, y = 5; side lengths: 45, 458. Determine whether the quadrilateral is a parallelogram. Explain.≅and ≅•Yes; if two opposite sides are congruent, then the quadrilateral is a parallelogram.•No; if the diagonals of a quadrilateral bisect each other, this is not enough to prove that the quadrilateral is a parallelogram.•No; if two opposite sides are congruent, this is not enough to prove that the quadrilateral is a parallelogram.•Yes; if the diagonals of a quadrilateral bisect each other, then the quadrilateral is a parallelogram.9. Can coordinate geometry be used to prove that opposite sides and in quadrilateral EFGH are congruent?•Yes; use the Distance Formula to show that the diagonals are congruent.•No; you can only show that EF is parallel to GH by using coordinate geometry.•Yes; use the Distance Formula between vertices E and F, and between vertices G and H.•No; you can only find the slopes of EF and GH by using coordinate geometry.10. A square WXYZ has the vertices W(b, b), X(b, –b), Y(–b, –b), and Z(–b, b). Which vertex is in QuadrantII?•W•Z•X•Y11. ∠J and ∠M are base angles of isosceles trapezoid JKLM. If m∠J = 21x + 4, m∠K = 12x– 8, and ∠M = 14x+ 10, find the value of x.•• 2•–•–912. Suppose you are using coordinate geometry to prove that quadrilateral WXYZ is a square. Explain why no twosides should be parallel to the y-axis.•The x-axis would intersect two sides of the square, so the coordinates of the corners would not be clear.•Sides which are parallel to the y-axis would have an undefined slope, so you cannot prove numerically that these sides are parallel.•Points on the sides which are parallel to the y-axis could have any y-value.•The sides of the square which are parallel to the y-axis could be easily confused with the y-axis.13. Find AM if PN = 8 and AO = 5.•8• 3•13• 514. Given square ABCD, where A = (0, a), B = (a, a), C = (0, 0), and D = (a, 0). To prove that the diagonal ADis times the length of side CD, first use _____ to find that = a and = a. Therefore, the ratio= , or .•the definition of isosceles triangle ACD•the definition of right angle C•the Distance Formula•the definition of the origin C = (0, 0)15. A farmer is building a fence for his yard. He is considering two designs, which are shown below. Explain whythe quadrilaterals formed by the horizontal rails and the slanting boards are parallelograms in both designs.•The horizontal rails are parallel to each other. A parallelogram has exactly one pair of parallel sides, so both quadrilaterals are parallelograms.•The horizontal rails are congruent to each other. The slanting boards are all congruent to each other. A parallelogram has two pairs of adjacent sides, but opposite sides are not congruent, so both quadrilaterals are parallelograms.•The horizontal rails are congruent to each other. The slanting boards are all congruent to each other. A parallelogram has four congruent sides, so both quadrilaterals are parallelograms.•The horizontal rails are parallel to each other. The identical slanting boards all slant at the same angle, so the sides are parallel. A parallelogram has both pairs of sides parallel, so both quadrilaterals are parallelograms.16. A rhombus is centered on the origin. One side of the rhombus goes through the points (a, 0) and (0, b). Whatare possible coordinates for one of the other sides?•(–a, 0), (a, 0)•(b, 0), (0, –a)•(0, –b), (0, b)•(–a, 0), (0, –b)17. If a quadrilateral is a parallelogram, then its opposite sides are _____.•perpendicular•adjacent•congruent•none of these18. Find the value of each variable in the parallelogram. m∠1 = 10x, m∠2 = x + y, and m∠3 = 18z.•x = 9, y = 81, z = 5•x = 18, y = 167, z = 5•x = 18, y = 162, z = 10•x = 9, y = 86, z = 019. Complete ≅ _____ for parallelogram EFGH. Then state a definition or theorem as the reason.•; because the angles of a parallelogram bisect each other•; because the diagonals of a parallelogram bisect each other•; because the diagonals of a parallelogram bisect each other•; because the angles of a parallelogram bisect each other20. Which of the following sets of points represents a line segment in Quadrant III and its reflection in the x-axis?(Use the positive numbers a, b, c, d for the coordinates of the endpoints).•(a, b), (c, d); reflection (–a, b), (–c, d)•(–a, –b), (–c, –d); reflection (–a, b), (–c, d)•(a, b), (c, d); reflection (a, –b), (c, –d)•(–a, –b), (–c, –d); reflection (a, –b), (c, –d)1. Solve for a and b.•a = , b =•a = , b =•a = , b =•a = , b =2. State whether ΔADB∼ΔCDB, and if so, identify the theorem that proves the triangles similar.•yes, SSS∼•yes, AA∼•yes, SAS∼•no3. ABCDE∼GHJDF. Complete the congruence and proportion statements.a.∠H≅b.=• a.B; b.AE• a.E; b.DC• a.E; b.AE• a.B; b.DC4. Write a similarity statement for the two triangles.•ΔVUT∼ΔWXY•ΔTVU∼ΔWXY•ΔTUV∼ΔWXY•ΔTUV∼ΔWYX5. Find the geometric mean of 48 and 3.•9•25.5•12•166. The extendable ramp shown below is used to move crates of fruit to loading docks of different heights. When thehorizontal distance AB is 4 feet, the height of the loading dock, BC, is 3 feet. What is the height of the loading dock, DE?•7 ft•9 ft•11 ft7. Find the geometric mean of 20 and 5.•10• 4•12.5•258. In movies and television, the ratio of the width of the screen to the height is called the aspect ratio. Televisionscreens usually have an aspect ratio of 4 : 3, while movie screens usually have an aspect ratio of 1.85 : 1. However, if a movie is made for television in "Letterbox" format, it retains the 1.85 : 1 aspect ratio and fills in the top and bottom parts of the screen with black bars. What would be the height of a movie in "Letterbox" format on a television screen that measures 25 inches along its diagonal? (Hint: First find the width and height of the television screen.)•13.51 in.•10.81 in.•15 in.•8.12 in.9. Use the diagram to determine the height of the tree.•264 ft•72 ft•60 ft•80 ft10. The two rectangles are similar.Which is a correct proportion between corresponding sides?•=•=•=11. Use the Side-Splitter Theorem to find x given that || .•18•12•24• 612. Find OM if bisects ∠NLM, LM = 14, NO = 3, and LN = 4. Round your answer to the nearest hundredth, ifnecessary.•12.27•18.67•0.86•10.513. There is a law that the ratio of the width to length for the American flag should be 10 : 19. Which dimensionsare NOT in the correct ratio?•20 by 38 in.•50 by 95 ft•20 by 44 ft•100 by 190 ft14. If one measurement of a golden rectangle is 6.8 inches, which could be the other measurement?•8.418 in.•11.002 in.• 1.618 in.• 5.182 in.15. If one measurement of a golden rectangle is 8.2 inches, which could be the other measurement?•9.818 in.• 6.582 in.• 1.618 in.• 5.068 in.16. Solve = .•20•19•15•2417. Find OM if bisects ∠NLM, LM =15, NO = 5, and LN = 11. Round your answer to the nearest hundredth, ifnecessary.•33• 6.82• 3.67•8.5918. The width of a golden rectangle is 3 m, which is shorter than the length. What is the length?• 1.85 m• 2.32 m• 3.64 m• 4.85 m19. Find and simplify the ratio of the length to the width of the rectangle.••••20. ΔBGH∼ΔSWQ. What are the pairs of corresponding sides?•BG and SQ, BH and SW, GH and WQ•BG and GB, SQ and QS, GH and HG•BG and SW, BH and SQ, GH and WQ•BG and WQ, BH and SW, GH and SQ1. A building near Atlanta, Georgia, is 181 feet tall. On a particular day at noon it casts a 204-foot shadow. Whatis the sun's angle of elevation at that time?•41.6°•27.5°•62.5°•48.4°2. In right triangle ΔABC, sin A = . What is cos A?••••none of these3. Find the ratio for cos x.••••14. Find the value of x to the nearest meter.•46 m•40 m•35 m•36 m5. Compare the quantity in Column A with the quantity in Column B. The diagram may not be drawn to scale.•The quantity in Column A is greater.•The quantity in Column B is greater.•The two quantities are equal.•The relationship cannot be determined on the basis of the information given.6. How many of these triples could be sides of a right triangle: (27, 36, 45), (12, 17, 20), (24, 32, 40), (14,48, 50)?• 4 triples• 3 triples• 2 triples• 1 triple7. Find the ratio for cos x.•••2•8. Find a third number of the Pythagorean triple that includes 72 and 75.•9•21•37•1049. Find the measure of the marked acute angle to the nearest degree.•62°•28°•61°•118°10. In ΔABC, ∠A is a right angle and m∠B = 60. If AB = 20 ft, find BC. If necessary, round your answer tothe nearest tenth.•10 ft.•40 ft•20 ft•ft11. Find the value of the variable to the nearest hundredth.• 5.28 cm•0.32 cm• 3.13 cm• 5.12 cm12. Find the length of the leg of the right triangle. Leave your answer in simplest radical form.•48••288•13. In ΔABC, ∠A is a right angle and m∠B = 45. If AB = 20 ft, find BC.•10 ft•20 ft•40 ft•20 ft14. Which direction bearing is shown?•19° north of east•19° north of west•19° south of east•19° south of west15. Leslie used the diagram to compute the distance from Ferris to Dunlap to Butte. How much shorter is the distancedirectly from Ferris to Butte than the distance Leslie found?•123 mi•87 mi•36 mi•84 mi16. Which vector has a direction of 31° east of north?••••17. Find the value of x to the nearest tenth.•14.4• 6.3•7.8• 3.118. Find the value of x.•3•6•12• 619. Find the value of x to the nearest tenth.•7.8•18.3•33.0•8.920. Find the value of x to the nearest integer when tan x = 1.483.•58•56•55•571. Which type of isometry is the equivalent of two reflections across two vertical lines?•translation•rotation•glide reflection•none of these2. A section of a tessellated plane is shown below. Which types of symmetry does the tessellated plane have?•glide-reflectional symmetry•translational, rotational, and glide-reflectional symmetry•rotational symmetry•translational and reflectional symmetry3. A blueprint for a house has a scale of 1 : 30. A wall in the blueprint is 7 in. What is the length of the actualwall?•210 ft•21 ft•17.5 ft•none of these4. What is the image of the point (4, –2) after a rotation 270° clockwise about the origin?•(–4, 2)•(4, 2)•(–2, 4)•(2, 4)5. Which graph shows a triangle and its reflection image in the x-axis?••••6. Write a rule to describe a reflection over the y-axis.•(x, y) → (–x, y)•(x, y) → (–x, –y)•(x, y) → (x, –y)•(x, y) → (y, x)•reflectional•rotational•rotational and reflectional•none of these8. Find the image of O(0, 0) after two reflections, first in y = 4, and then in x = –7.•(7, –4)•(–14, 8)•(8, –14)•(–4, 7)9. A section of a tessellated plane is shown below. Which types of symmetry does the tessellated plane have?•rotational symmetry•translational and rotational symmetry•reflectional symmetry•glide-reflectional symmetry•rotational•reflectional•rotational and reflectional•none of these11. Describe the translation 7 units to the left, 12 units up using a vector.•)–12, 7*•)12, –7*•)–7, 12*•)7, –12*12. If the figure has rotational symmetry, find the angle of rotation about the center that results in an imagethat matches the original figure.•120°•90°•72°•It has no rotational symmetry.13. Find the glide reflection image of the solid triangle for the translation )–6, –3* and reflection in y = –1.••••14. If a point P(1, –2) is reflected across the line x = 3, what are the coordinates of its reflection image?•(1, –4)•(1, 8)•(–7, –2)•(5, –2)15. Which translation from thin-lined figure to thick-lined figure is given by the vector )6, 6*?••••16. The dotted triangle is a dilation image of the solid triangle. What is the scale factor?•• 2• 3•17. Find the image of C under the translation described by each vector.a.)4, 5*b.)11, –8*• a.A; b.B• a.B; b.A• a.E; b.D• a.D; b.E18. What is the image of the point (–3, 4) after a rotation of 90° counterclockwise about the point (–3, 0)?•(–7, 0)•(1, 0)•(0, –3)•(–3, –4)19. Which letter has rotational symmetry?• E•X•J•T20. Use scalar multiplication to find the image of the quadrilateral for a dilation with center (0, 0) and scalefactor 2. Graph the quadrilateral and its image.••••。
加拿大高中考英语试卷

一、听力(共20分,每题2分)1. How are you doing today?A. I'm fine, thank you.B. I'm not good, I have a headache.C. I'm doing great, I just finished a big project.2. What time is it now?A. It's 8:00 am.B. It's 9:00 am.C. It's 10:00 am.3. Where is the nearest bank?A. It's on the corner of the street.B. It's across the street.C. It's next to the park.4. How do you get to the library?A. By bus.B. By subway.C. By walking.5. What is your favorite food?A. Pizza.B. Pasta.C. Sushi.二、阅读理解(共30分,每题3分)阅读以下短文,回答问题。
My favorite place is the beach. It's a beautiful place with golden sand and clear blue water. I love to go there with my family during the summer vacation. We spend the whole day at the beach, swimming, sunbathing, and playing beach volleyball. The sound of the waves and the cool breeze make me feel relaxed and happy. When I'm at the beach, Ifeel like I'm in a different world.6. What is the author's favorite place?A. The park.B. The beach.C. The mountain.7. What does the author do at the beach during the summer vacation?A. Go shopping.B. Go sightseeing.C. Go swimming, sunbathing, and playing beach volleyball.8. Why does the author like the beach?A. Because it's beautiful.B. Because it's a place to relax.C. Both A and B.三、完形填空(共20分,每题2分)阅读以下短文,从每题的四个选项中选择最佳答案。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
加拿大安大略省十年级数学试卷-英文2 of 11GRADE 10 PRINCIPLES OF MATHEMATICS (ACADEMIC)MPM 2DTotal Marks:INSTRUCTIONS:1. Calculators may be used.2. Read all instructions carefully in order to maximize your mark.A/C[K]Part A – Multiple Choice 25 Marks (25 questions * 1 mark each)For each of the following questions in this section, circle the letter representing the correct answer.1. A linear system of two equations that has one solution represents two lines that are:a)parallel b)coincident c)intersecting d)none of these2.The midpoint of RS is M(8, -1). If point S has coordinates (11, 4) what are the coordinates of point R?a)(3, -6)b)(15, -6)c)(5, -6)d)(3, 9)3.The midpoint of the line segment with end points A(-8, 8) and B(6, 4) is:a)(0, 10)b)(1, 2)c)(7, 2)d)(-1, 6)4.The equation of a horizontal line passing through the point (4, 2) is:a)b)c)d)5.The equation of a line with a slope of and a y intercept of 8 is:a)b)c)d)2=x 4=y 2=y 4=x 5=m 85+=x y 85+-=x y 85--=x y 58+=xy3 of 116.The slopes of 2 lines are -7 and. These lines are said to be:a)parallel b)perpendicular c)coincident d)none of these7.The slope of a line segment passing through 2 points (10,- 4) and (-2, -16) is:a)1b)2c)-1d)-28.The length of a line segment with end points (-6, 7) and (-1, -5) is:a)12b)5c)13d)1699.The diameter of a circle whose equation is is:a)15b)16c)17d)none of these10.The equation of a circle with a centre of (0, 0) that also passes through the point (-8, -6) is:a)b)c)d)11.The y-intercept of the line is:a)2b)-2c)10d)512.The slope of the line is:a)2b)-2c)1d)013.If (-3, y) is a solution to the equation , what is the value of y?a)3b)6c)5d)814.The product is equal to:a)b)c)d)15.A simplified expression for is:a)b)c)d)7128922=+y x 1022=+y x 10022=+y x 1422=+y x 4822=-y x 01052=+-y x 0124=-+y x 132=+y x ()()z y x z y x 323243--2612z xy 26412z y x 2612z xy -00412z y x ()()n m n m ----52m 7n m 27+m 3-nm 27-4 of 1116.A simplified expression for is:a)b)c)d)17.The slope of the line, which is perpendicular to the line, is:a)-4b)4c)1d)-118.The shortest distance from the point (2, -3) to the line is:a)5b)3c)2d)619.The value of the polynomial when is:a)59b)44c)13d)2920.Which of the following is not a function:a)b)c)d)21.The range of the relation whose equation is is:a)b)c)d)22.The vertex of the parabola is:a)b)c)d)23.The equation of the axis of symmetry of the parabola is:a)b)c)d)24.A parabola with a vertex of and a stretch factor of (relative to ) would have an equation of:a)b)c)d)25The parabola passes through the point . The value of is:242927abc bc a --ac 3abc 323ac 223c a 084=+-y x 4-=x 8542+-a a 3-=a ()()(){}7,6,5,4,3,222x y =22y x =()()(){}3,8,3,7,2,652--=x y 5-≤y 5≤y 5-≥y 5≥y ()642--=x y ()6,4-()6,4-()4,6-()4,6-()5242+--=x y 5=x 5-=x 2=x 2-=x ()3,241-2x y =()32412+--=x y ()32412++-=x y ()23412-+-=x y ()23412++-=x y k x y +-=24()3,2-k5 of 11a)-19b)11c)13d)19A/C Part B – Short AnswersFor each of the questions in this section, write your answers in the spaces provided.Use the foolscap provided for any rough work. Show details of calculationswherever requested.1.In the accompanying diagram, state each of the following: (4 Marks)[K]a)domain: __________ (1 Mark)[K]b)range: __________ (1 Mark)[C]c)Is the relation a function? Justifyyour answer. (2 marks)[A] 2.The x-intercepts of the parabola are: __________ and__________.(Show your work) (2 Marks)[A] 3.The roots of the quadratic equation are: __________ and__________.(Show your work) (3 Marks)2892-=x y 0101732=+-x x 24-2-46 of 11x[A] 4.Write the equation of the parabola with a vertex of (4, 23) if it passes through thepoint (-1, -2): (Show your work) (3 Marks)____________________[T] 5. A line passes through 2 points (1, 4) and (2,-4). Calculate the slope of the line. Alsoshow the equation of the line in the form . (Show your work) (4 Marks)________________________________________Slope Equation[K] 6.The Tangent of is: __________ (1 Mark)[A]7.a) In the accompanying diagram, the two triangles are similar. What is the value of?(Show your work) (2 Marks)__________[T]b) If the area of the smaller triangle is 8 cm 2, what is the area of the largertriangle?(Show your work) ( 2 Marks)Area = __________[K]8Given that sin A = , find (to the nearest degree) __________ (1 Mark)0=++C By Ax 45x =x 21A ∠1127 of 11[A]9.In the accompanying right triangle, find the value of x to one decimal place.(Show your work) (2 Marks)________[A]e the SINE LAW to find the value of side x to one decimal place.(Show your work) (2 Marks)x = ________[A]e the COSINE LAW to find the value of side x to one decimal place.(Show your work) (2 Marks)x = ________[T]12.Factor each of the following to the fullest extent possible: (4 Questions * 2 marks each)a)________________________b)________________________=x y x my mx 22--+31142--x x 328︒x56︒42︒x3056︒2030x8 of 11c)________________________d)________________________A/C Part C – Full Solutions RequiredFor each of the questions in this section, full solutions are required. Record your answers in the spaces provided. Use the foolscap provided for any rough work.[A] 1.Solve the linear system using the elimination method. Remember to find valuesfor both x and y. (5 Marks)[C]Explain what the solution above represents geometrically. How do you knowthat the solution you arrived at is the correct answer? (2 Marks)[A] 2.Expand and simplify the polynomial . (4 Marks)2416916y x -2225309s rs r +-225=+y x 2132-=-y x ()()()21432+-+-x x x9 of 11[T] 3.Find the equation of the line perpendicular to the line and passingthrough the point (-4, 1). (4 Marks)[T] 4.From the window of one building, a man finds that the angle of elevation to thetop of a second building is 47︒ and the angle of depression to the bottom of thesame building is 58︒. The buildings are 60 m apart. Find the height of the 2ndbuilding to the nearest metre. A diagram is required. (6 Marks)[T] 5.∆ABC has vertices A(1, 7), B(-5, 3) and C(3, -1). Determine the equation for AE,the altitude from vertex A to the opposite side BC. (5 Marks)088=-+y x6.The hypotenuse of a right triangle is 26 cm. The sum of the other two sides is 34cm. (9 Marks)[T]a)Find the length of the other two sides of the triangle. (3 Marks)[T]b)Find the measure of the other two angles. Round to the nearest degree. (3 Marks)[C]c)Describe a situation where you would be able to use knowledge of thePythagorean theorem in a practical, real life situation. (3 Marks)10 of 1111 of 11[T]7. A rectangular skating rink measures 20m by 20m. It has been decided toincrease the area of the rink by a factor of 4. Determine how much each side should be extended. Assume that each side is extended by the same amount. (6 Marks)[C]What is the significance of keeping the skating rink in the shape of a square?Justify your answer. (3 Marks)[A]8.a)Solve using the quadratic formula. (2 Marks)[A]b)Solve by factoring. Check your solutions. (2 Marks)35122+=d d 03122=-x。
