Structural Fluctuations in the Spin Liquid State of Tb2Ti2O7
第八届国际凝聚态理论与计算材料学会议

大会将请专家对以下领域作专题性的特邀报告:
(1). 凝聚态物理的最新进展:
A. 自旋电子学
B. 纳米材料
C. 固体量子信息和计算
D. 玻色-爱因斯坦凝聚
E. 强关联电子系统
F. 高温超导
G. 量子霍尔效应
H. 磁学
I 表面和界面
J. 半导体物理
K. 低维凝聚态物理
L. 介观物理
M. 软凝聚态物质
N. 生物物理
structure Metallic Phases in Solid Germane (GeH4) under Pressure 锗烷在
压力下的金属相
11:45 -12:15
12:15 -13:00
午餐
张振宇 橡树岭国家实验室
柳百新
清华大学
林海青 香港中文大学 牛谦 美国德克萨斯大学
7 月 16 日
凝聚态理论专题邀请报告 I 主持人:谢心澄 地点:芙蓉厅
14:30 -14:55 14:55 -15:20 15:20 -15:45 15:45 -16:10 16:10 -16:35 16:35 -16:50
7 月 16 日
Manipulating Magnetization States of Nanostructures Two-spin decoherence in semiconductor quantum dots
稀磁半导体(Al,Cr)N 中的氮空位与高温铁磁性的第一原理研究
蒋青 刘邦贵
吉林大学 中科院物理所
15:20 -15:45 15:45 -16:10 16:10 -16:35
Theoretical and experimental studies of semiconductor dilute nitrides and devices
融合基因分析方法在肿瘤研究中的应用与发展

【摘 要】 融合基因是指两个独立基因的编码区首尾相连所形成的且置于同一套调控序列控制的产物,研究表明许多癌症的发生与融合基因存在密切的联系。
融合基因可作为癌症治疗的靶点,在癌症诊断及治疗领域中融合基因的研究具有重要意义。
部分融合基因驱动癌症的机制已被初步揭示,但是有些真实存在的在肿瘤发生发展过程中有重要意义的融合基因由于工具和实验技术限制还未被发现。
因此,对融合基因的分析预测研究方法逐渐成为关注的热点之一。
本文探讨了目前常用的关于融合基因的分析工具及方法,为融合基因在癌症中的研究提供思路。
【关键词】 融合基因;分析工具;检测;验证DOI:10. 3969 / j. issn. 1000-8535. 2023. 08. 001Development and application of fusion gene analysis methods in tumors researchZHANG Junwei,GUAN XinchaoSchool of Public Health,Guangzhou Medical University【Abstract】 Fusion genes are the products of two independent genes whose coding regions are linked and controlled by the same set of regulatory sequencesused as targets for cancer therapythe mechanisms of fusion genes driving cancer have been initially revealedthe process of tumor development have not been discovered due to the limitation of tools and experimental techniquesthe analysis and prediction methods of fusion genes are becoming a hot topic of interestanalytical tools and methods on fusion genes to provide ideas for the study of fusion genes in cancer【Key words】 fusion gene现代研究普遍认为,肿瘤的发生与遗传物质的改变密切相关。
heterogeneous interfacial structure

heterogeneous interfacial structure英文版Heterogeneous Interfacial StructureHeterogeneous interfacial structure refers to the structural differences that exist at the boundary between two different materials or phases. This structure plays a crucial role in determining the physical and chemical properties of the interface, as well as its stability and reactivity.At the interface between two materials, the atomic arrangement, bonding configuration, and electronic structure can all differ significantly from the bulk materials on either side. This heterogeneity can lead to a range of unique properties, such as charge accumulation, bond formation, and catalytic activity. For example, in the field of materials science, heterogeneous interfaces are often exploited to enhance the performance of devices such as solar cells and fuel cells.The study of heterogeneous interfacial structure is challenging due to the complexity of the interactions involved. Experimental techniques such as scanning probe microscopy, spectroscopy, and diffraction methods can provide insights into the atomic-scale structure and electronic properties of interfaces. Computational modeling is also an important tool for understanding and predicting interfacial behavior.In recent years, there has been increasing interest in the use of heterogeneous interfacial structures in nanotechnology and materials science. This interest is driven by the potential for novel materials with enhanced properties, as well as the development of new technologies such as nanodevices and sensors.In conclusion, heterogeneous interfacial structure is a crucial aspect of materials science and nanotechnology. Its understanding and control offer the potential for the development of novel materials and devices with enhanced performance and functionality.中文版异质界面结构异质界面结构指的是两种不同材料或相之间的边界处存在的结构差异。
交流散热风扇内部结构

交流散热风扇内部结构The inner workings of an AC cooling fan are a marvel of simplicity and efficiency. It's fascinating to think that these unassuming devices, often tucked away in our electronics, play such a crucial role in keeping things running smoothly. Let's delve into the heart of these little dynamos and explore the intricate dance of components that brings about the cooling breeze. At the coreof every AC cooling fan lies the motor, the driving force behind the fan's operation. This electric motor, typically an induction motor, converts electrical energy into mechanical energy, setting the stage for the fan's rotational movement. The motor consists of two key parts: the stator and the rotor. The stator, a stationary component, houses coils of wire that generate a rotating magnetic field when an alternating current flows through them. This rotating magnetic field then interacts with the rotor, a freely rotating component within the stator. The interaction induces currents in the rotor, creating its own magnetic field. The interplay of these magnetic fields results in a torque that sets the rotor spinning. Attached to the rotor is the fan blade, the component responsible for generating the airflow. These blades, often crafted from plastic or metal, are meticulously designed to optimize air movement. The shape, angle, and number of blades all contribute to the fan's performance, influencing factors like airflow volume, air pressure, and noise level. As the rotor spins, driven by the motor,the blades cut through the air, creating a current that draws in air from the surroundings and propels it outward. This continuous flow of air serves todissipate heat from the components it's directed towards. The motor and fan blade assembly is housed within a frame, providing structural support and protection. The frame material can vary depending on the fan's application and operating environment. Common materials include metal, plastic, and even specialized composites for demanding situations. The frame not only holds the components together but also often features mounting points, allowing the fan to be securely attached to its designated location within a device. To ensure smooth andefficient operation, bearings play a crucial role. These small but mighty components reduce friction between the rotating rotor and the stationary frame. By minimizing friction, bearings enable the rotor to spin freely with minimalresistance, enhancing the fan's longevity and reducing noise generation. Various types of bearings are employed in AC cooling fans, each with its own set of characteristics. Sleeve bearings, known for their simplicity and cost-effectiveness, are often found in less demanding applications. Ball bearings, on the other hand, offer greater durability and smoother operation, making them suitable for high-performance scenarios. The electrical connection to the AC cooling fan is established through wires that supply power to the motor. These wires are typically color-coded to indicate their function, facilitating proper connection and ensuring safe operation. The voltage and current requirements of the fan are carefully matched to the power source, ensuring compatibility and preventing damage. Beyond these core components, AC cooling fans may incorporate additional features tailored to specific applications. Some fans include speed control mechanisms, allowing for adjustment of the airflow based on cooling needs. Others may integrate temperature sensors, enabling the fan to automatically regulate its speed in response to temperature fluctuations. These advanced features enhance the fan's versatility and efficiency, making them adaptable to a wide range of operating conditions. The seemingly simple act of generating a cooling breeze is, in reality, a testament to the ingenuity of engineering. The interplay of electrical and mechanical components, meticulously designed and assembled, results in a device that plays a vital role in countless applications. From keeping our computers humming along to ensuring the smooth operation of industrial equipment, AC cooling fans stand as unsung heroes, quietly and effectively dissipating heat and maintaining the delicate balance of temperature that keeps our world running.。
海港码头混凝土表面氯离子随高度变化规律

Industrial Construction Vol.40,No.7,2010工业建筑2010年第40卷第7期75海港码头混凝土表面氯离子随高度变化规律*胡狄赵羽习龚奇鹤金伟良(浙江大学土木工程学院,杭州310029)摘要:海水中氯离子的侵蚀是影响钢筋混凝土结构在海洋环境中耐久性的一个重要的因素,而混凝土表面氯离子浓度是研究氯离子侵蚀的重要指标之一。
由于混凝土结构沿海拔不同高度的服役环境不同,表面氯离子浓度随高程是变化的,但其分布存在一定的规律。
根据浙江东部沿海地区某混凝土码头结构表面氯离子浓度的实测数据,通过数据统计分析,提出表面氯离子浓度随高度呈单峰高斯分布的规律;通过建立BP 神经网络预测模型,验证其高斯分布规律假设的合理性。
在对浙东沿海某混凝土码头及日本不同海域混凝土码头的表面氯离子浓度实测数据进行概率统计分析后,得到沿海混凝土结构的最严重氯离子侵蚀区域为海拔在0.4 1.6m ,其海水浸润时间比为0.285 0.478,应重点加强该区域结构的抗氯侵蚀能力。
最后,通过对实测数据的归一化处理,建立了基于高斯函数的海港码头混凝土表面氯离子浓度的高度影响系数和海水浸润时间比影响系数经验表达式。
关键词:混凝土结构;表面氯离子浓度;海拔高度CONCRETE SURFACE CHLORIDE ION CONCENTRATION VARYING WITH HEIGHT IN MARINE ENVIRONMENTHu DiZhao YuxiGong QiheJin Weiliang(Department of Civil Engineering ,Zhejiang Uinversity ,Hangzhou 310029,China )Abstract :Chloride ion is the main reason that impacts the durability of reinforced concrete structures in marine environment ,while the surface chloride concentration of concrete structures is one of the most important factor influencing the transport process of chloride into structural concrete.Because of the different local environments for concrete structures at different heights ,the surface chloride concentration varys with the heights.Based on the inspected data of surface chloride concentration from a concrete port which locates at eastern coastal area of Zhejiang Province ,a single peak Gaussian distribution is proposed to describe the surface chloride concentration along the height of structure.A model of surface chloride using BP neural network is established to predict the distribution of the surface chloride concentration along the height.To find out the most serious chloride aggression region of reinforced concrete structures ,probability based statistical analysis of inspection data from concrete ports of eastern coastal area of Zhejiang Province and Japan is carried out.The most serious chloride aggression height is from 0.4to 1.6m above the sea level ,from 0.285to 0.478of water infiltration time ratio ,where the anti-aggression capacity of chloride should be strengthened.Finally ,according to the normalized inspected data ,empirical equations are proposed to describe the influence of height and water infiltration time on surface chloride ion concentration based on a Gaussian Function.Keywords :concrete structures ;surface chloride concentration ;height*国家自然科学基金重点项目(50538070);国家自然科学基金项目(50808157);交通部西部交通建设科技项目(200631822302-06);国家科技支撑计划课题(2006BAJ03A03-03)。
Astructurefordeoxyribosenucleicacid
Complexity: The sequence of bases on one strand of DNA determines the sequence on the potential strand through the principle of completeness This means that the order of bases on one strand is complete to the order on the other strand, with A pairing with T and G pairing with C
Structural constraint elements
The structural constraint elements of DNA include the nucleotides, which are made up of a phase group, a deoxyribose sugar, and a nitrogen base (A, T, G, or C)
In addition to the nucleotides, DNA also contains epigenetic marks such as methylation and acetylation, which can affect gene expression without altering the DNA sequence itself
Fluid-Structure Interaction

Fluid-Structure Interaction Fluid-Structure Interaction (FSI) is a complex and interdisciplinary fieldthat involves the interaction between a fluid flow and a solid structure. This interaction can have significant effects on the behavior and performance of both the fluid and the structure, making it a crucial consideration in various engineering applications. The study of FSI is essential in understanding phenomena such as flutter in aircraft wings, vortex-induced vibrations in offshore structures, and blood flow in arteries. One of the key challenges in FSI is accurately modeling and simulating the interaction between the fluid and the structure. This requires a deep understanding of both fluid dynamics andstructural mechanics, as well as sophisticated computational tools to solve the coupled equations governing the FSI problem. The development of numerical methods for FSI simulations has been a major focus of research in recent years, with the goal of improving the accuracy and efficiency of FSI models. In addition to numerical simulations, experimental techniques play a crucial role in studying FSI phenomena. Physical testing allows researchers to validate and calibrate their numerical models, as well as to investigate complex FSI behaviors that aredifficult to capture through simulations alone. Experimental facilities such as wind tunnels, water tanks, and biomedical labs provide valuable data for understanding the dynamics of FSI and for developing new design guidelines for engineering structures. The study of FSI has wide-ranging applications across various industries, including aerospace, civil engineering, biomechanics, and marine engineering. In aerospace, FSI is essential for predicting the aerodynamic performance and structural integrity of aircraft components, such as wings and fuselage. In civil engineering, FSI is used to analyze the behavior of buildings and bridges under wind and earthquake loads, as well as to optimize the design of offshore structures for oil and gas production. From a biomedical perspective, FSI is critical for understanding the flow of blood in arteries and theinteraction between blood vessels and surrounding tissues. This knowledge is essential for diagnosing and treating cardiovascular diseases, as well as for designing medical devices such as stents and artificial heart valves. In marine engineering, FSI is used to study the response of ships and offshore platforms towaves and currents, as well as to optimize the design of marine structures for improved performance and safety. Overall, the study of Fluid-StructureInteraction is a fascinating and challenging field that requires amultidisciplinary approach to address complex engineering problems. By combining theoretical analysis, numerical simulations, and experimental testing, researchers can gain valuable insights into the behavior of fluid-structure systems and develop innovative solutions for a wide range of practical applications. The continued advancement of FSI research will lead to new discoveries and technologies that will shape the future of engineering and science.。
风能的利用英语作文

风能的利用英语作文The Untapped Potential of Wind EnergyIn a world grappling with the pressing issue of climate change, the search for sustainable and renewable energy sources has become increasingly paramount. Among the various alternatives to traditional fossil fuels, wind energy has emerged as a promising solution, offering a clean, abundant, and cost-effective means of power generation. As the global community strives to reduce its carbon footprint and transition towards a greener future, the utilization of wind energy holds the key to unlocking a more sustainable energy landscape.The fundamental principle behind wind energy is the conversion of the kinetic energy present in wind into electrical energy. This process is facilitated by wind turbines, which harness the power of the wind to spin their blades, thereby driving a generator that produces electricity. The beauty of this technology lies in its simplicity and its ability to be deployed on a large scale, making it a scalable and versatile solution for energy production.One of the primary advantages of wind energy is its renewable and inexhaustible nature. Unlike fossil fuels, which are finite resources that contribute to the depletion of the Earth's natural reserves, wind is a perpetual and self-replenishing source of energy. As long as the Earth's atmospheric conditions continue to generate wind, the potential for wind-powered electricity generation remains virtually limitless. This characteristic ensures that wind energy can be relied upon as a sustainable energy source for generations to come, reducing our dependence on finite and environmentally damaging fossil fuels.Moreover, the environmental benefits of wind energy are undeniable. Unlike traditional power plants that rely on the combustion of fossil fuels, wind turbines generate electricity without producing any direct greenhouse gas emissions or other pollutants. This clean and emission-free nature of wind energy aligns perfectly with the global efforts to mitigate the impacts of climate change and reduce the carbon footprint of energy production. By embracing wind energy, we can play a crucial role in transitioning towards a more environmentally responsible and sustainable energy landscape.In addition to its environmental advantages, wind energy also offers significant economic benefits. The installation and operation of wind farms have the potential to create numerous job opportunities,ranging from the manufacturing and construction of turbines to the ongoing maintenance and management of wind farms. This economic stimulus can be particularly valuable in rural or remote areas, where wind resources are often abundant, but other forms of economic development may be limited. Furthermore, the decreasing costs of wind energy technology and the increasing efficiency of wind turbines have made wind power increasingly cost-competitive with traditional energy sources, making it an attractive investment for both individuals and governments.Despite the numerous benefits of wind energy, its widespread adoption is not without challenges. One of the primary obstacles is the intermittent nature of wind, which can lead to fluctuations in power generation. The variability of wind speeds can create challenges in maintaining a consistent and reliable supply of electricity, particularly when wind energy is integrated into existing power grids. To address this issue, researchers and engineers are exploring innovative solutions, such as energy storage technologies, to ensure a more stable and consistent supply of wind-generated electricity.Another challenge lies in the potential environmental and social impacts of large-scale wind farm development. The construction and operation of wind turbines can have implications for local wildlife, particularly birds and bats, which may be affected by collisions orhabitat disruption. Additionally, the visual and noise impacts of wind farms can be a concern for nearby communities, leading to potential conflicts and resistance to their development. To mitigate these challenges, wind energy projects must be carefully planned and implemented with a focus on minimizing environmental and social impacts, through measures such as site selection, turbine design, and community engagement.Despite these challenges, the potential of wind energy remains immense, and its role in the global energy landscape is poised to grow significantly in the coming years. As governments and policymakers around the world recognize the urgency of addressing climate change and the need for sustainable energy solutions, wind power is increasingly becoming a priority in energy policy and investment decisions.In conclusion, the utilization of wind energy represents a crucial step towards a more sustainable and environmentally responsible future. By harnessing the power of the wind, we can generate clean, renewable electricity, reduce our reliance on fossil fuels, and contribute to the global effort to mitigate the impacts of climate change. While challenges exist, the benefits of wind energy are clear, and with continued research, innovation, and policy support, the untapped potential of this renewable resource can be fully realized,paving the way for a cleaner, greener, and more sustainable energy future for all.。
蛋白质的三维结构

Chapter 04 The Three Dimensional Structure of Proteins第四章蛋白质的三维结构1. Properties of the Peptide Bond(肽键的性质)InX-ray studies of crystalline peptides, Linus Pauling and Robert Corey found that the C—N bond in the peptide link is intermediate in length (1.32 A) between a typical C—N single bond (1.49 Å) and a C=N double bond (1.27 Å). They also found that the peptide bond planar (all four atoms attached to the C—N group are located in the same plane) and that the two α-C atoms attached to the C—N are always trans to other (on opposite sides of the peptide bond): 在晶体肽X-射线衍射研究中,Linus Pauling 和 Rbert Corey发现,在肽键中C-N键的键长介于典型的C-N单键(1.49 Å)和C=N双键(1.27 Å)之间。
他们还发现,肽键是平面的(与C-N相连的所有四个原子在同一个平面内),与C-N相连的两个α-C 原子总是互为反式的(在肽键的相对面)(a) What does the length of the C—N bond in the peptide linkage indicate about its strength and its bond order (i.e., whether it is single, double, or triple)? 肽键中C-N键的键长说明其强度和键级(单、双或三键)的什么情况?(b) What do the observations of Pauling and Corey tell us about, the ease of rotation about the C—N peptide bond? Linus Pauling 和 Rbert Corey的观察告诉我们C—N肽键转动的什么情况?2. Structural and Functional Relationships in Fibrous Proteins(纤维状蛋白结构与功能的关系) William Astbury discovered that the X-ray pattern of wool shows a repeating structural unit spaced about 5.2 Å along the direction of the wool fiber. When he steamed and stretched the wool, the x-ray pattern showed a new repeating structural unit at a spacing of 7.0 Å. Steaming and stretching the wool and then letting it shrink gave an x-ray pattern consistent with the original spacing of about 5.2 Å. Although these observations provided important clues to the molecular structure of wool, Astbury was unable to interpret them at the time. William Astbury 发现,羊毛的X-射线衍射谱表明在羊毛的纤维方向有间距大约5.2 Å的重复结构单位。
Kagome Spin Liquid戈薇的自旋液体

Second Renormalization
Kagome
h (corr) 12 0.081
S 1S 2l1b ˆl2c ˆ
0.005 S1S2l1l2
- 0.039 S1 S2 ly1ly2
0.112 S1 S2
0.1 S 1S 2l 2b ˆ l 1c ˆ
n,n ~n
~n Z 1n P n n'n ~n' ~n' n
Step I: Divide lattice to disjoint blocks. Diagonalize H on each Block.
block excitations are the ''atoms'' (composite particles)
Truncate: M lowest states per block
• The triangular lattice flows to chiral symmetry breaking, probably the 3 sublattice Neel phase.
• Future: Investigations of the quantum phase transition in the effective Hamiltonian by following the RG flow.
Effective Bond Interactions
h( corr) 12
0.953
S 1S 2l1b ˆl2c ˆ
0.2111 S1S2l1l2
0.053 S1 S2 ly1ly2
0.1079 S1 S2
结构化学 英语

结构化学英语Structured ChemistryChemistry is a vast and complex field of study that encompasses the understanding of the composition, structure, and properties of matter. One of the key aspects of chemistry is the concept of structure, which plays a crucial role in determining the behavior and characteristics of chemical substances. Structural chemistry, a subfield of chemistry, focuses on the spatial arrangement of atoms and molecules, and how this arrangement influences the chemical and physical properties of materials.The study of structure in chemistry involves the investigation of the three-dimensional (3D) arrangements of atoms within molecules and the intermolecular interactions that exist between them. This knowledge is essential for understanding the behavior of chemical systems, predicting their properties, and designing new materials with desired characteristics.One of the fundamental tools used in structural chemistry is X-ray crystallography. This technique involves the bombardment of a crystalline sample with X-rays, which interact with the electrons inthe atoms of the crystal. The resulting diffraction pattern can be analyzed to determine the precise arrangement of atoms within the crystal structure. This information is crucial for understanding the properties of solid-state materials, such as metals, minerals, and ceramics.Another important technique in structural chemistry is nuclear magnetic resonance (NMR) spectroscopy. This method utilizes the magnetic properties of atomic nuclei to provide information about the chemical environment and connectivity of atoms within a molecule. NMR spectroscopy is widely used in the identification and characterization of organic compounds, as well as in the study of biomolecules, such as proteins and nucleic acids.In addition to these experimental techniques, computational methods have also become increasingly important in the field of structural chemistry. Quantum mechanical calculations, such as density functional theory (DFT), allow researchers to model the behavior of atoms and molecules at the quantum level, providing insights into their electronic structure and chemical reactivity.One of the key applications of structural chemistry is in the design and development of new materials. By understanding the relationship between the structure of a material and its properties, chemists can engineer substances with specific characteristics, suchas high strength, enhanced thermal stability, or improved electrical conductivity. This knowledge is particularly valuable in fields like materials science, nanotechnology, and catalysis.Another important aspect of structural chemistry is its role in the study of biological systems. The structures of proteins, nucleic acids, and other biomolecules are crucial for understanding their functions and interactions within living organisms. This knowledge is essential for the development of new drugs and the understanding of disease processes.In conclusion, the field of structural chemistry is a fundamental and multifaceted discipline that underpins our understanding of the physical and chemical properties of matter. Through the use of advanced experimental and computational techniques, structural chemists continue to unravel the mysteries of the molecular world, paving the way for new discoveries and innovations that have the potential to transform our lives.。
Fluid-Structure Interaction and Dynamics

Fluid-Structure Interaction and Dynamics Fluid-structure interaction (FSI) is a complex and fascinating field that involves the interaction between fluid flow and solid structures. This interaction can have significant impacts on the behavior and performance of various engineering systems, ranging from aircraft wings to cardiovascular stents. Understanding and predicting the dynamics of FSI is crucial for designingefficient and reliable systems. One of the key challenges in FSI is accurately modeling the behavior of both the fluid and the structure. Fluid flow is typically described by the Navier-Stokes equations, which govern the motion of viscous fluids. On the other hand, the structure is often modeled using finite element analysis, which represents the solid deformations under external forces. Combining these two models to simulate the interaction between the fluid and the structure requires sophisticated numerical techniques and computational tools. The dynamics of FSI can be influenced by a variety of factors, such as the geometry of the structure, the properties of the fluid, and the boundary conditions. For example, the shape and flexibility of an aircraft wing can affect its aerodynamic performance, while the viscosity and density of the fluid can impact the flow patterns around the wing. Additionally, the boundary conditions at the interface between the fluid and the structure play a crucial role in determining the overall behavior of the system. In the context of biomedical engineering, FSI plays a critical role in understanding the behavior of blood flow in arteries and veins. Cardiovascular diseases, such as atherosclerosis, can alter the flow patterns in blood vessels, leading to potentially life-threatening conditions. By simulating the FSI dynamics in blood vessels, researchers can gain insights into the underlying mechanisms of these diseases and develop new treatment strategies. Despite the challenges and complexities associated with FSI, advancements in computational fluid dynamics (CFD) and structural analysis have enabled researchers to make significant progress in this field. High-performance computing resources and advanced simulation techniques have made it possible to simulate complex FSI problems with a high degree of accuracy and reliability. These simulations can provide valuable insights into the behavior of engineering systems and help optimize their design and performance. In conclusion, fluid-structureinteraction and dynamics are essential components of many engineering systems, with applications ranging from aerospace to biomedical engineering. By understanding and predicting the complex interactions between fluids and structures, researchers and engineers can design more efficient and reliable systems. Continued advancements in computational tools and simulation techniques will further enhance our ability to study and optimize FSI dynamics, leading to innovative solutions and breakthroughs in various industries.。
Control of matter defects using optical phase singularities
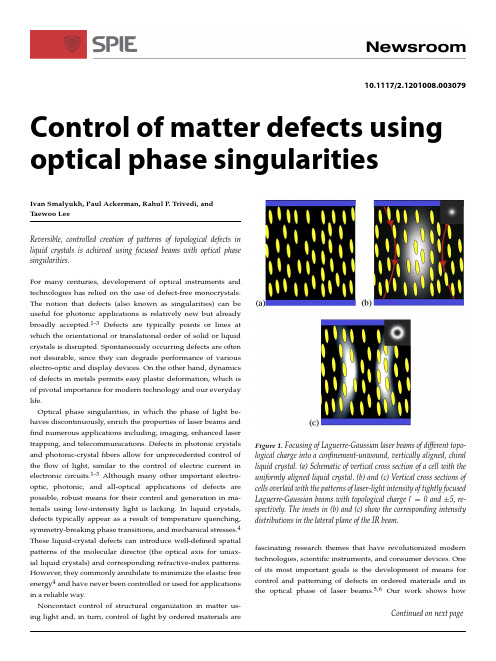
10.1117/2.1201008.003079 Control of matter defects using optical phase singularitiesIvan Smalyukh,Paul Ackerman,Rahul P.Trivedi,and Taewoo LeeReversible,controlled creation of patterns of topological defects in liquid crystals is achieved using focused beams with optical phase singularities.For many centuries,development of optical instruments and technologies has relied on the use of defect-free monocrystals. The notion that defects(also known as singularities)can be useful for photonic applications is relatively new but already broadly accepted.1–3Defects are typically points or lines at which the orientational or translational order of solid or liquid crystals is disrupted.Spontaneously occurring defects are often not desirable,since they can degrade performance of various electro-optic and display devices.On the other hand,dynamics of defects in metals permits easy plastic deformation,which is of pivotal importance for modern technology and our everyday life.Optical phase singularities,in which the phase of light be-haves discontinuously,enrich the properties of laser beams and find numerous applications including,imaging,enhanced laser trapping,and telecommunications.Defects in photonic crystals and photonic-crystalfibers allow for unprecedented control of theflow of light,similar to the control of electric current in electronic circuits.1–3Although many other important electro-optic,photonic,and all-optical applications of defects are possible,robust means for their control and generation in ma-terials using low-intensity light is lacking.In liquid crystals, defects typically appear as a result of temperature quenching, symmetry-breaking phase transitions,and mechanical stresses.4 These liquid-crystal defects can introduce well-defined spatial patterns of the molecular director(the optical axis for uniax-ial liquid crystals)and corresponding refractive-index patterns. However,they commonly annihilate to minimize the elastic free energy4and have never been controlled or used for applications in a reliable way.Noncontact control of structural organization in matter us-ing light and,in turn,control of light by ordered materialsare Figure1.Focusing of Laguerre-Gaussian laser beams of different topo-logical charge into a confinement-unwound,vertically aligned,chiral liquid crystal.(a)Schematic of vertical cross section of a cell with the uniformly aligned liquid crystal.(b)and(c)Vertical cross sections of cells overlaid with the patterns of laser-light intensity of tightly focused Laguerre-Gaussian beams with topological charge l D0and˙5,re-spectively.The insets in(b)and(c)show the corresponding intensity distributions in the lateral plane of the IR beam.fascinating research themes that have revolutionized modern technologies,scientific instruments,and consumer devices.One of its most important goals is the development of means for control and patterning of defects in ordered materials and in the optical phase of laser beams.5,6Our work shows howContinued on next pageFigure 2.Optical generation of arbitrary spatial patterns of Torons.(a)Schematic visualization of the optical generation of a Toron using a laser beam with optical phase singularity.(b)Different types of Toron structures formed by focusing Laguerre-Gaussian laser beams of differ-ent topological charge.2The largest Toron defect structure is approxi-mately 15 m in diameter.(c)Optically generated periodic 2D pattern of Torons with a dislocation.Each Toron is 10 m in diameter.(d)Characters obtained by Toron generation,each approximately 5 m in diameter.(e)Square-periodic pattern of Torons generated in a liquid-crystal sample.The Torons are each 5 m in diameter.laser beams with optical phase singularities can be used to control topological singularities in ordered,liquid-crystalline materials,6potentially enabling a number of new applications.We employed a computer-controlled,phase-only,spatial-light modulator (SLM,Boulder Nonlinear Systems)to generate holo-grams and convert an IR Gaussian beam into doughnut-shaped Laguerre-Gaussian laser beams of different topological charge (defining the number of twists that the phase of the light makes in one wavelength).6We then focused the beams into the bulk of the untwisted chiral liquid crystal confined between thin glass plates:see Figure 1(a)–(c).These chiral liquid crystals have a strong preference for molecular twisting,but can be untwisted by external fields and confinement,as shown in Figure 1(a).Us-ing focused Gaussian and Laguerre-Gaussian vortex laser beams with different optical phase singularities—see Figure 1(b)and (c)—we generated topological liquid-crystal defect architectures containing both point and line singularities:see Figure 2(a).6The defects are bound to each other by twisted interdefect re-gions,forming stable or metastable 3D configurations.In chiral nematic liquid crystals that are confined into sandwich-like cells with vertical boundary conditions,these laser-generated topo-logical defects embed the localized 3D twist into the uniform background of the director field.They are untwisted becauseof confinement,forming distinct localized chiro-elastic particle-like excitations of different types:see Figure 2(a)and (b).6These defect structures—dubbed ‘Torons’6—can be generated at a desired location in the sample and their internal structures can then be controlled by varying the topological charge of the Laguerre-Gaussian laser beam.The resultant Torons are com-prised of topological point-and ring-shaped defects of opposite topological charge,such that the overall charge is conserved:see Figure 2(a)and (b).6We also find that vortex laser beams of power 10–100mW with screw-dislocation defects in the optical phase allow for control of the topological defects and internal configurations of Torons at a desired spatial location,enabling formation of desired long-term-stable defect superstructures.We show three examples of such superstructures containing Torons of dif-ferent kinds,a square-periodic lattice with a dislocation in Figure 2(c),a structure in the form of the characters ‘SPIE’in Figure 2(d),and a regular periodic pattern:see Figure 2(e).Us-ing both single-beam steering and holographic laser-intensity patterning,7the periodic crystal lattices of Torons can be generated and tailored by tuning their periodicity,reorienting their crystallographic axes,introducing dislocation defects in the periodic patterns,etc.These periodic lattices can be dynamically modified,erased,and then recreated,depending on the need of the relevant application.Periodicity of these optically induced structures depends on the equilibrium pitch of the chiral ne-matic liquid crystal,and it can be tuned from several hundreds of nanometers to hundreds of microns by varying the pitch and using different structure-generation schemes.The key advantage of our approach is the robustness with which the periodic patterns of liquid-crystal defects can be generated and switched between multiple distinct states.6The unprecedented control over organization of the defects offers promise for a wide range of applications,such as optical-data storage,light/voltage-controlled information displays,and tunable photonic crystals.Our preliminary results show that they can be used as efficient optically reconfigurable diffrac-tion gratings.The structural multistability as well as low-voltage and low-laser-power switching may lead to powerless and low-power multimodal operation of electro-optic,all-optical,and information display devices.Our future work will be directed at realizing these applications,so that the optically con-trolled defects in liquid crystals may find use in controlling the properties of light.Continued on next pageThis work was supported by the Renewable and Sustainable Energy Initiative and Innovation Initiative Seed Grant Programs of the University of Colorado at Boulder,the International Institute for Complex Adaptive Matter,and by National Science Foundation (NSF)grants (DMR-0820579,DMR-0844115,DMR-0645461,and DMR-0847782).Paul Ackerman was supported by the NSF-funded JILA-Physics Research Experience for Undergraduates program at the University of Colorado.Author InformationIvan SmalyukhUniversity of Colorado at Boulder Boulder,COIvan Smalyukh is an assistant professor of physics,a founding fellow of the Renewable and Sustainable Energy Institute,and a senior investigator of the Liquid Crystal Materials Research Center.His research interests are at the interface of op-tics/photonics,soft-condensed-matter physics,nanoscience,and renewable energy.Paul Ackerman,Rahul P .Trivedi,and Taewoo Lee Department of PhysicsUniversity of Colorado at Boulder Boulder,COPaul Ackerman is an undergraduate research assistant in Smalyukh’s research group.His research interests include liquid crystals,laser trapping and manipulation,and tunable diffrac-tion gratings.Rahul Trivedi is a PhD student and a research assistant in Smalyukh’s group.His research interests include laser trapping and manipulation,nonlinear optical microscopy,liquid-crystal defects,optical generation of topological defects and structures,and electro-optics of liquid crystals.Taewoo Lee is a postdoctoral research associate.His research interests focus on the development of novel optical-imaging techniques,such as coherent anti-Stokes Raman-scattering polarizing microscopy,multiphoton-excitation fluorescence mi-croscopy,second-harmonic-generation polarizing microscopy,and imaging of liquid crystals,polymers,colloids,and biomole-cular materials.References1.M.Qi,E.Lidorikis,P .T.Rakich,J.D.Joannopoulos,E.P .Ippen,and H.I.Smith,A three-dimensional optical photonic crystal with designed point defects ,Nature 429,pp.538–541,2004.2.C.H.Sun and P .Jiang,Acclaimed defects ,Nat.Photon.2,pp.9–11,2008.3.K.Ishizaki and S.Noda,Manipulation of photons at the surface of three-dimensional photonic crystals ,Nature 460,pp.367–370,2009.4.P .M.Chaikin and T.C.Lubensky,Principles of Condensed Matter Physics ,Cambridge Univ.Press,2000.5.J.Leach,M.R.Dennis,J.Courtial,and M.J.Padgett,Laser beams:knotted threads of darkness ,Nature 432,p.165,2004.6.I.I.Smalyukh,nsac,N.Clark,and R.Trivedi,Three-dimensional structure and multistable optical switching of Triple Twist Toron quasiparticles in anisotropic fluids ,Nat.Mater.9,pp.139–145,2010.7.S.Anand,R.P .Trivedi,G.Stockdale,and I.I.Smalyukh,Non-contact optical con-trol of multiple particles and defects using holographic optical trapping with phase-only liquid crystal spatial light modulator ,Proc.SPIE 7232,p.723208,2009.c2010SPIE。
r 克拉夫结构动力学英文版

r 克拉夫结构动力学英文版R Cranefly Structural Dynamics - English VersionIntroduction:Structural dynamics is a vital field of study in engineering, focusing on the response and behavior of structures under dynamic loading conditions. Understanding the dynamic characteristics of structures is crucial for ensuring their stability, integrity, and performance. This article aims to provide an overview of the R Cranefly software package, which offers powerful tools for analyzing structural dynamics, and its key features in the English language version.1. Overview of R Cranefly:R Cranefly is a comprehensive software package developed for conducting structural dynamics analysis. It enables engineers and researchers to simulate and predict the behavior of structures under a variety of dynamic loading conditions. With its user-friendly interface and extensive capabilities, R Cranefly has become a go-to tool for professionals working in the field of structural dynamics.2. Key Features of R Cranefly:2.1. Time Domain Analysis:R Cranefly facilitates time domain analysis, allowing users to examine the structural response and behavior over time. This feature enables the evaluation of dynamic effects such as transient vibrations, oscillations, and resonance phenomena. By analyzing the time domain responses, engineerscan accurately assess the performance and stability of structures under dynamic loading.2.2. Frequency Domain Analysis:The software also offers frequency domain analysis, which involves investigating the structural response in the frequency spectrum. By applying Fourier analysis, R Cranefly enables the identification of dominant frequencies, resonance effects, and modal characteristics. This feature proves invaluable in designing structures to withstand specific dynamic loads.2.3. Modal Analysis:Modal analysis is a crucial technique for identifying and quantifying the natural modes of vibration in a structure. R Cranefly provides a comprehensive suite of tools for conducting modal analysis, including methods such as the finite element method. Engineers can extract mode shapes, natural frequencies, and damping ratios, which aid in understanding the dynamic behavior of structures.2.4. Response Spectrum Analysis:R Cranefly supports response spectrum analysis, which is an effective method for evaluating structural responses to seismic excitations. This analysis enables engineers to design structures to withstand earthquake forces by considering the expected ground motion and the inherent characteristics of the structure.3. R Cranefly User Interface:The user interface of R Cranefly is designed with an emphasis on usability and efficiency. Its intuitive layout enables users to define structural properties, load inputs, and analysis parameters seamlessly. Additionally, the software provides detailed graphical outputs, including displacement, acceleration, and response spectra plots, aiding in the interpretation of structural responses.4. Applications of R Cranefly:R Cranefly finds applications across a wide range of sectors, including civil engineering, aerospace, mechanical engineering, and architecture. It proves invaluable in the design and assessment of buildings, bridges, dams, aircraft, and other structures subjected to dynamic loading. With its versatility and extensive capabilities, R Cranefly has become a preferred choice for professionals involved in structural dynamics analysis.Conclusion:R Cranefly, with its diverse set of analysis tools, intuitive interface, and comprehensive capabilities, is an invaluable resource for conducting structural dynamics analysis. By accurately assessing the response and behavior of structures under dynamic loading, engineers can design safe, efficient, and durable structures. The English version of R Cranefly opens up new possibilities for international users to explore and utilize this powerful software package in their work.。
fluctuation

fluctuationFluctuation: Understanding the Nature and Impact of VariabilityIntroduction:Fluctuation refers to the unpredictable and continuous change in a system, variable, or process. It is a natural phenomenon found in various aspects of our lives, from the fluctuations in weather patterns to economic markets, and even in our personal experiences. Understanding the nature and impact of fluctuations is essential as it allows us to make informed decisions, adapt to changes, and plan for the future. This document explores the concept of fluctuation, its causes, effects, and ways to manage its impact.Understanding Fluctuation:Fluctuation is an inherent characteristic of many systems and processes, often resulting from multiple interconnected factors. It can be observed in various domains, including science, finance, and human behavior. In scientific contexts, fluctuations can occur at the atomic and molecular level, leading to variations in temperature, pressure, or other measurable quantities. In financial markets, fluctuations in stock prices, exchange rates, and commodity prices arecommon occurrences that impact investors and economies. Similarly, fluctuations in human emotions, productivity, and even natural populations are subjects of interest in psychology and ecology.Causes of Fluctuation:Fluctuations can be caused by a variety of factors, both internal and external to the system or process under consideration. Internal factors include inherent variability in the system, such as random events, quantum effects, or intrinsic volatility. External factors may involve changes in environmental conditions, market trends, technology advancements, or social and political factors. Fluctuations can also emerge from the interaction between multiple variables or systems, creating complex and often unpredictable patterns.Effects of Fluctuation:Fluctuations can have significant effects on various aspects of our lives. In economic systems, fluctuations in markets can result in booms and recessions, affecting businesses, employment, and overall economic stability. In climate systems, fluctuations in temperature and weather patterns can lead to extreme events like storms, heatwaves, or droughts, impacting agriculture and human settlements. Fluctuations in populations, such as disease outbreaks orinvasive species introductions, can have far-reaching consequences for ecosystems and biodiversity.Managing the Impact of Fluctuation:While fluctuations are inevitable, there are strategies and approaches to manage their impact. In financial markets, investors and traders employ risk management techniques, diversify their portfolios, and use statistical models to predict and mitigate the effects of fluctuations. In climate science, advanced monitoring systems and predictive models help policymakers and communities prepare for extreme weather events and plan adaptation measures. In business and personal life, understanding the cyclical nature of fluctuations can help individuals make informed decisions and develop resilience to navigate uncertain times.Conclusion:Fluctuations are an intrinsic part of our world, occurring in various systems and processes around us. Understanding the causes and effects of fluctuations is crucial for adaptability and decision-making. By recognizing the patterns, managing risks, and planning for potential impacts, we can harness the power of fluctuations and turn them into opportunities for growth and innovation. Whether it is in financial markets, climate systems, or our personal lives, embracing andmanaging fluctuations becomes an essential skill to thrive in an ever-changing world.。
生物大分子结构与功能第14章纤维蛋白剖析

第十四章: 纤维蛋白
fibrous proteins
主动脉
腱
Three most abundant residues in fibrous proteins
存在于丝毛中的a螺旋纤维是有柔性的,它们可以伸展为近两倍的长度, 并且具有弹性以使得当张力被释放时可以恢复到原始的长度。胶原蛋白纤维与之 相比则更具有强度抗拉伸,因而相对具有刚性β折叠片纤维。既具有强度也有柔 性最好的例子是蜘蛛网纤维,某些蜘蛛的网比相同粗细的钢丝还要强,并且同时 还具有柔性,以使得在蛛网上捕捉到昆虫时不被弄破。纤维β折叠片在细胞内也 可由一些可溶性球蛋白的错误折叠产生因而会引起,像阿尔茨海默Alzheimer’s 病和疯牛病prion等疾病。
3, Elastin (弹性蛋白): in ligaments and arterial blood vessels
(韧带)
(动脉血管)
4, Fibroin (丝心蛋白): in silk made by silkworms or spiders
Fibrous Proteins: Structural Materials of Cells and Tissues
蛋白质通常可分为两种截然不同的功能类型,即由排列 为长的蛋白纤维所构成的被动的结构材料和排列为小的致密结 构域的多肽链所组成的细胞机理的活性成分。不管它们在结构 和功能上的差异如何,这些类型的蛋白都含有由环区域所隔开 的a螺旋和β折叠片。在大多数情况下,纤维蛋白含有特殊的重 复氨基酸顺序,这对于它们的特殊三维结构来说是必需的。
结构生物学英语

结构生物学英语One of the key techniques used in structural biology is X-ray crystallography. This method involves growing crystals of the macromolecule of interest, which can then be bombarded with X-rays. The X-rays interact with the atoms in the crystal and create a diffraction pattern, which can be used to determine the positions of the atoms in the macromolecule. This information can then be used to build a three-dimensional model of the macromolecule.Another important technique in structural biology is nuclear magnetic resonance (NMR) spectroscopy. In NMR, a strong magnetic field is used to align the spins of atomic nuclei in the macromolecule. By applying radiofrequency pulses and measuring the resulting signals, information about the distances and angles between different atoms in the macromolecule can be obtained. This data can then be used to generate a three-dimensional model of the macromolecule.Structural biologists use the atomic models generated from these experiments to gain insights into how proteins, nucleic acids, and other macromolecules function in cells. By understanding their structure, scientists can infer how they interact with other molecules, how they carry out their biological functions, and how they can be targeted with drugs to treat diseases.One area of focus in structural biology is the study of membrane proteins. These proteins are embedded in the lipid bilayer of cell membranes and play crucial roles in cell signaling, transport of molecules across membranes, and many other processes. Determining the structures of membrane proteins is challenging, as they are difficult to crystallize and are often unstable outside the lipid bilayer. However, recent advances in cryo-EM have enabled researchers to determine the structures of many membrane proteins, providing valuableinsights into their function.。
结构振动与动态子结构方法书英文

结构振动与动态子结构方法书英文Structural Vibrations and Dynamic Substructuring Methods.Structural vibrations are a fundamental aspect of many engineering disciplines, ranging from civil engineering to aerospace applications. These vibrations can be caused by various external forces such as wind, earthquake, or machine operations. Understanding and predicting these vibrations is crucial for ensuring the safety, efficiency, and durability of structures.Dynamic substructuring methods are a set of techniques used to analyze complex structures by dividing them into smaller, more manageable substructures. This approach allows for efficient numerical modeling and simulation of vibrations, particularly in large-scale systems where traditional methods may be computationally intensive.Basics of Structural Vibrations.Structural vibrations occur when a structure is subjected to external forces that cause it to move or deform. These forces can be periodic, such as those caused by rotating machinery, or non-periodic, such as those resulting from earthquakes. The response of the structure, including its displacement, velocity, and acceleration, is dependent on its mass, stiffness, and damping characteristics.The natural frequencies and mode shapes of a structure are key parameters in understanding its vibration behavior. Natural frequencies represent the resonant frequencies at which the structure tends to vibrate, while mode shapes describe the pattern of vibration at each frequency. These parameters can be obtained through modal analysis, which involves exciting the structure and measuring its response.Dynamic Substructuring Methods.Dynamic substructuring methods are based on the principle of modal synthesis. Instead of modeling theentire structure as a single, complex system, the structure is divided into smaller substructures, each with its own set of natural frequencies and mode shapes. These substructures are then coupled together to form the complete structure.One of the most commonly used dynamic substructuring methods is the fixed-interface modal synthesis. In this approach, the substructures are assumed to have fixed interfaces with each other, and the vibrations at these interfaces are used to couple the substructures. This allows for efficient modeling of the overall structure by reducing the number of degrees of freedom required for analysis.Another popular method is the free-interface modal synthesis, where the substructures are allowed to have free interfaces. This approach provides more flexibility in modeling the interactions between substructures but requires more complex coupling techniques.Applications of Dynamic Substructuring.Dynamic substructuring methods find applications in various engineering fields. In civil engineering, they are used to analyze the vibrations of bridges, buildings, and towers. In aerospace engineering, these methods are employed to study the dynamic behavior of aircraft and spacecraft. In mechanical engineering, dynamic substructuring is used to model and simulate the vibrations of machines and components.The key advantage of dynamic substructuring is its efficiency. By dividing a complex structure into smaller substructures, it becomes easier to model and analyze each substructure separately. This reduces the computational requirements and allows for faster simulation of theoverall structure. Additionally, the method provides a modular approach to modeling, where new substructures can be easily added or replaced without affecting the existing model.Challenges and Future Directions.Despite its advantages, dynamic substructuring methods also face some challenges. One of the main challenges is the accurate modeling of interface interactions between substructures. Achieving accurate coupling requires careful consideration of the boundary conditions and interface dynamics.Another challenge is the scalability of these methods to even larger and more complex structures. As the size and complexity of structures increase, so does the computational demand for analysis. Future research in dynamic substructuring could focus on developing more efficient algorithms and optimization techniques to handle these larger systems.In conclusion, structural vibrations and dynamic substructuring methods play a crucial role in understanding and predicting the dynamic behavior of structures. By dividing complex structures into manageable substructures, these methods enable efficient modeling and simulation, leading to safer, more efficient, and durable structures. Future research and advancements in this field willcontinue to push the boundaries of structural analysis and design.。
First-principles study of the structural, vibrational, phonon and thermodynamic
1. Introduction Ultra-high temperature ceramics (UHTCs) with melting temperatures in excess of 3000 K are usually composed by the refractory borides, carbides and nitrides of early transition metals [1–7]. Among the UHTCs, transition metal carbides (TMC) such as TiC, ZrC and HfC are metallic compounds with unique physical and chemical properties including an extremely high melting point and hardness, chemical stability, corrosion resistance combined with metallic electrical and thermal conductivities [5–10]. These features give transition metal carbides the capability to withstand high temperatures in oxidizing environments, making them candidates for applications in the atmosphere of extreme thermal and chemical environments [6,7]. The structural, vibrational, phonon and thermodynamic properties of IVb group transition metal carbides have been investigated experimentally [10–17] and theoretically [13,18–28] in the earlier reports. In the 1970s, the phonon dispersion relations of TiC, ZrC and HfC were measured using inelastic neutron scattering by Pintschovius et al. [10] and Smith et al. [15–17]. Lattice dynamics calculation and the phonon dispersion relations of transition metal carbides such as ZrC and HfC were reported using a phenomenological ‘‘double-shell’’ model theory [18] where long-range interatomic interactions were taken into account in order to get a
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :0707.1682v 1 [c o n d -m a t .d i s -n n ] 11 J u l 2007Structural Fluctuations in the Spin Liquid State of Tb 2Ti 2O 7J.P.C.Ruff,1B.D.Gaulin,1,2J.P.Castellan,1K.C.Rule,1J.P.Clancy,1J.Rodriguez,1and H.A.Dabkowska 11Department of Physics and Astronomy,McMaster University,Hamilton,Ontario,L8S 4M1,Canada 2Canadian Institute for Advanced Research,180Dundas St.W.,Toronto,Ontario,M5G 1Z8,Canada High resolution X-ray scattering measurements on single crystal Tb 2Ti 2O 7reveal finite structural correlations at low temperatures.This geometrically frustrated pyrochlore is known to exhibit a spin liquid,or cooperative paramagnetic state,at temperatures below ∼20K.Parametric studies of structural Bragg peaks appropriate to the Fd ¯3m space group of Tb 2Ti 2O 7reveal substantial broadening and peak intensity reduction in the temperature regime 20K to 300mK.We also observe a small,anomalous lattice expansion on cooling below a density maximum at ∼18K.These measurements are consistent with the development of fluctuations above a cooperative Jahn-Teller,cubic-tetragonal phase transition at very low temperatures.PACS numbers:75.25.+z,75.40.Gb,75.40.-s,78.70.ckA magnetic material whose lattice geometry prevents the simultaneous satisfaction of the local magnetic inter-actions is said to be geometrically frustrated.Such ma-terials,many of which are based on triangular and tetra-hedral crystalline architectures,are of intense current in-terest because they have a natural proclivity towards ex-otic quantum mechanical ground states [1].As the lead-ing magnetic interactions on such lattices are frustrated,rather subtle subleading terms in the Hamiltonian,such as interactions beyond nearest-neighbours,weak disor-der,and the order-by-disorder mechanism,tend to de-termine the ultimate ground state of the material.The rare-earth titanates,with formula A 2Ti 2O 7,are a well-studied family of frustrated magnetic insulators.In this family,the A-site is occupied by a trivalent rare-earth ion with eight-fold oxygen coordination,while the Ti 4+ion has six-fold oxygen coordination.Both the A 3+site and the Ti 4+site independently reside on the py-rochlore structure,a face-centered cubic lattice of corner-sharing tetrahedra which is a playground for phenomena related to geometrical frustration.Depending on the nature of the magnetic rare-earth ion in these materi-als,their ground states can exhibit long-range magnetic order [2,3],spin ice physics [4,5],and in the case of Tb 2Ti 2O 7,a highly correlated quantum disordered state known as a collective paramagnet or spin liquid [6,7,8].The low temperature properties of Tb 2Ti 2O 7have been extensively studied.However,the crystal lattice is generally assumed to be a passive bystander to the spin liquid physics.In this letter,we present high res-olution x-ray scattering evidence for strong fluctuations in the low temperature lattice of Tb 2Ti 2O 7,signifying both a feedback of frustration onto the crystal lattice,and the importance of the lattice degrees of freedom in determining the exotic ground state of this material.Current interest in Tb 2Ti 2O 7is largely due its failure to attain magnetic order of any kind to tem-peratures as low as 20mK,despite having an antifer-FIG.1:(H,K,0)reciprocal space maps of structural Bragg peaks of Tb 2Ti 2O 7.(a)(12,0,0)at T=40K.(b)(12,0,0)at T=0.3K.(c)(8,8,0)at T=40K.(d)(8,8,0)at T=0.3K.Logitudinal (arrowhead)and transverse directions in recipro-cal space are indicated in each panel.The low temperature broadening of the Bragg peaks is preferentially longitudinal for (12,0,0)and preferentially transverse for (8,8,0).romagnetic (AFM)Curie-Weiss constant of ∼18K [6,7,8].Magnetic neutron scattering on Tb 2Ti 2O 7at low temperatures[8]shows diffuse scattering indicative of spin correlations over the spatial extent of a single tetrahedron (∼3.6˚A )in zero magnetic field and am-bient pressure.When perturbed with either relatively weak magnetic fields or applied pressures,or both,long range magnetic order can be induced at temperatures of ∼1-3K [9,10,11].Low lying crystal field levels for Tb 3+in this environ-ment have been measured[12]and they are consistent with local [111]anisotopy,such that Tb moments are constrained to point into or out of the tetrahedra.How-ever,the diffuse magnetic neutron scattering[7]as wellas the presence ofdispersive spin waves in the magneticfield-induced ordered state[9]of Tb2Ti2O7at low tem-peratures are more easily understood in terms of con-tinuous spin symmetries.This quandry has been been recognized and discussed for some time[13,14].Much earlier magneto-elastic measurements on Tb2Ti2O7revealed both giant magnetostriction[15]and an anomolous Young’s modulus[16]at low tempera-tures,indicating the importance of the coupling be-tween magnetic and lattice degrees of freedom.Strong magnetoelastic effects have also been topical in cubic spinel antiferromagnets.The spinels have chemical com-position AB2O4,and the B-sublattice ions also reside on a network of corner-sharing tetrahedra;that is the pyrochlore lattice.Stronglyfirst order structural andmagnetic phase transitions can occur simultaneously in some of these materials at low temperatures.In the case of ZnCr2O4this occurs near12.5K,wherein the low temperature lattice undergoes a tetragonal distor-tion[17,18].Diffuse quasi-elastic magnetic scattering due to dynamic,short range spin correlations charac-teristic of geometrical frustration is associated with the high temperature cubic phase.This quasi-elastic mag-netic scattering becomes gapped within the low temper-ature tetragonal phase of ZnCr2O4,drawing similarities to spin-Peierls physics,albeit in three dimensional mate-rials.Other spinels exhibit unexpected plateaus in their magnetization as a function offield at low temperature which have been of intense interest[19,20].Such mag-netization plateaus have been modeled with biquadratic exchange terms in the effective spin Hamiltonian of these materials,arising from strong magneto-elastic coupling [21].Theoretically,spin-Peierls-like distortions of the pyrochlore lattice which act to better satisfy frustrated AFM interactions have been predicted[22].No such transition has been observed in Tb2Ti2O7despite sev-eral systematic studies[23,24].Large single crystals of Tb2Ti2O7and Y2Ti2O7were grown at McMaster University using the traveling sol-ventfloating zone technique,and a two-mirror,NEC image furnace.Both crystals were grown at a rate of 6mm/h in an Ar environment.The growth protocol was similar to that originally reported for Tb2Ti2O7 [25].Samples with aproximately cubic dimensions and 1mm3volumes were subsequently cut from the larger growths for use in the x-ray measurements.The samples were aligned with their(H,K,0)plane coincident with the scattering plane,and placed in a Janis3He cryostat mounted within a four-circle x-ray diffractometer.Cu Kα1radiation from an18kW rotating anode x-ray gen-erator was selected using a perfect single crystal germa-nium(110)monochromator.The diffracted beam was measured using a Bruker Histar area detector,mounted on the scattering arm at a distance of0.7m from the FIG.2:Cuts through the Bragg peaks,at both high and low temperatures,along withfits(solid lines)of the data to resolution convoluted Lorentzians,as described in the text. Intensities have been normalized to highlight the change in width.Top:Longitudinal broadening of the(12,0,0)peak. Bottom:Transverse broadening of the(8,8,0)peak. sample.This configuration allows for high-resolution characterization of Bragg peaks from room temperature down to∼300mK,with a temperature stability of∼10mK.Measurements were carried out on both Tb2Ti2O7 and Y2Ti2O7as a function of temperature for two high-angle Bragg reflections in the(H,K,0)plane,the(12, 0,0)and(8,8,0)Bragg peaks.Representative two-dimensional maps at T=40K and T=0.3K are shown in Fig. 1.The longitudinal and transverse(relative to the relevant reciprocal lattice vector)directions in recip-rocal space are indicated by the arrows and straight lines, respectively,superposed on thefigure.At low temper-atures,the peak intensities of both reflections are sup-pressed and the width of the scattering substantially in-creases as compared to the same data at40K.A careful inspection of the data shows that the(12,0,0)Bragg peak broadens preferentially in the longitudinal(H,0,0) direction,while the(8,8,0)reflection broadens preferen-tially in the transverse(H,-H,0)direction.In each case, the integrated intensity of the peaks in Fig.1shows no temperature dependence.Cuts through these maps at T=20K and T=0.3K are shown in Fig.2,highlighting the dominant broadening for each peak.In both cases,the peak intensities have been normalized and it is clear that the peak widths in-crease substantially as the temperature is lowered into the spin liquid regime appropriate to Tb2Ti2O7.Sim-ilar high resolution x-ray scattering measurements were carried out on the non-magnetic pyrochlore Y2Ti2O7, which shows no corresponding anomalous broadening orBragg intensity variation.We conclude that Tb2Ti2O7 developsfinite spatial lattice correlations below∼20K. We will argue that this is consistent with a system ap-proaching a cooperative Jahn Teller phase transition-albeit at unobtainably low temperatures.The top panel of Fig.3shows the temperature de-pendence of the peak intensity at the(12,0,0)and(8, 8,0)Bragg positions in Tb2Ti2O7.The temperature dependence at the(12,0,0)Bragg position in Y2Ti2O7 is also shown for comparison.Both Bragg intensities in Tb2Ti2O7decrease by about20%between20K and0.3 K,and the decrease is continuous.No corresponding ef-fect is seen in Y2Ti2O7.The data shown in Figs.1and 2was alsofit to an Ornstein-Zernike form[26]for spatial correlations(Eq.1)in order to extract inverse correla-tion lengths along both the longitudinal and transverse directions.S(q)=Aκ)2(1)Here,κis the inverse correlation length in the appro-priate direction.The data wasfit using resolution con-volution techniques wherein the T=40K peak served as the resolution function.Inverse correlation lengths ex-tracted from this analysis along the longitudinal(open symbols)and transverse(closed symbols)directions in reciprocal space are shown for the(12,0,0)and(8,8,0)Bragg peaks of Tb2Ti2O7in the middle and bottom panels of Fig.3,respectively.Two features are clear from the measured inverse cor-relation lengths.First,the structure departs from long range order at temperatures as high as20K,and spatial correlations diminish continuously as the temperature is lowered to∼0.3K.At the lowest temperatures,the spatial correlations still comprise many(∼80)unit cells. Nevertheless,the increase in peak width indicating the reduction in correlation length is clear(see Fig.2).Sec-ond,the broadening of the Bragg peaks is anisotropic. The longitudinal inverse correlation length at the(12, 0,0)Bragg position and T=0.3K is a factor of∼2.5 greater than the transverse inverse correlation length. At the(8,8,0)Bragg position,the anisotropy is re-versed with a larger inverse correlation length along the transverse direction at the lowest temperatures.The anisotropy in the inverse correlation lengths at both the(12,0,0)and(8,8,0)Bragg positions in Tb2Ti2O7is qualitatively consistent with cubic-to-tetragonal structuralfluctuations preceding a coopera-tive Jahn-Teller phase transition.A non-zero tempera-ture cubic-to-tetragonal structural transition would in-troduce a second,distinct lattice parameter into the structure.This would result in the(12,0,0)recipro-cal lattice vector(with|Q|=12×2πc)splitting in the longitudinal direction in reciprocal space.Recipro-cal lattice vectors of the form 8,8,0 would also show some splitting in the longitudinal direction,although it would be smaller than that at the(12,0,0)Bragg posi-tion by a factor of∼3.The principal splitting at the(8, 8,0)Bragg position would be expected in the transverse direction.TbVO4and TbAsO4show related behavior near and below their tetragonal-to-orthorhombic cooperative Jahn-Teller phase transitions at33.1K and29.0K, respectively[27,28].Similar high resolution x-ray scat-tering measurements performed with the same experi-mental set up on these two materials show an extreme form of this pattern of splitting of Bragg peaks below T C[29].These materials undergo the tetragonal-to-orthorhombic transition with twinning,such that the splitting of the(12,0,0)Bragg peak is exclusively lon-gitudinal,while that of the(8,8,0)Bragg peak is exclu-sively transverse.In addition,in a critical temperature regime above T C,Bragg peaks which ultimately split below T C exhibit the same type of broadening(longitu-dinal for Bragg peaks of the form(H,0,0)and trans-verse for Bragg peaks of the form(H,H,0)).This is indicative of structuralfluctuations above the coopera-tive Jahn-Teller phase transition.The anisotropic natureFIG.4:Relative change in lattice constant as a function of temperature in both Tb2Ti2O7and Y2Ti2O7.Inset:Focus on the anomalous behaviour within the spin liquid regime of Tb2Ti2O7.of both the broadening(above T C)and splitting(be-low T C)of the Bragg peaks is more extreme for TbVO4 and TbAsO4than for the case of the correlation lengths shown in Fig.3for Tb2Ti2O7.This is because the high temperature phase in TbVO4and TbAsO4is tetragonal rather than cubic,and measurements can focus on the unique(H,K,0)plane.Our high resolution x-ray measurements also allow an accurate determination of the lattice parameter in Tb2Ti2O7as a function of temperature.This is shown in Fig.4,along with the corresponding behavior of the lattice parameter in Y2Ti2O7.From room tempera-ture to∼18K,we observe the normal thermal con-traction of the lattice in both Tb2Ti2O7and Y2Ti2O7 .Interestingly,below∼18K,the Tb2Ti2O7lattice undergoes anomolous expansion which it maintains to our base temperature of∼0.3K.The inset to Fig.4 highlights the regime of thermal expansion with decreas-ing temperature at low temperatures.In contrast,the lattice parameter of non-magnetic Y2Ti2O7is indepen-dent of temperature below20K,as is typical for most materials.While the unusual expansion of the lattice parameter at low temperatures in Tb2Ti2O7is small(∆a[11]I.Mirebeau,I.N.Goncharenko,G.Dhalenne and A.Revcolevschi,Phys.Rev.Lett.93,187204(2004). [12]M.J.P.Gingras,B.C.den Hertog,M.Faucher,J.S.Gard-ner,S.R.Dunsiger,L.J.Chang,B.D.Gaulin,N.P.Raju, and J.E.Greedan,Phys.Rev.B,62,6496(2000). [13]M.Enjalran and M.J.P.Gingras,Phys.Rev.B,70,174426(2004).[14]H.R.Molavian,M.J.P.Gingras,and B.Canals,Phys.Rev.Lett.,98,157204(2007).[15]I.V.Aleksandrov,B.V.Lidskii,L.G.Mamsurova,M.G.Neigauz,K.S.Pigal’skii,K.K.Pukhov,N.G.Trusevich and L.G.Scherbakova,JETP62,1287(1985).[16]L.G.Mamsurova,K.S.Pigal’skii and K.K.Pukhov,JETP Lett.,43,755(1986).[17]S.H.Lee,C.Broholm,T.H.Kim,W.RatcliffII,andS.W.Cheong,Phys.Rev.Lett.84,3718-3721(2000).[18]S.-H.Lee,C.Broholm,W.Ratcliff,G.Gasparovic,Q.Huang,T.H.Kim,and S.-W.Cheong.Nature418,856-858(2002).[19]H.Ueda,H.A.Katori,H.Mitamura,T.Goto and H.Takagi.Phys.Rev.Lett.94,047202(2005).[20]M.Matsuda,H.Ueda,A.Kikkawa,Y.Tanaka,K.Kat-sumata,Y.Narumi,T.Inami,Y.Ueda and S.-H.Lee.Nature Physics Letters doi:10.1038/nphys586(2007). [21]Karlo Penc,Nic Shannon,Yukitoshi Motome and Hi-royuki Shiba.J.Phys.:Condens.Matter19145267 (2007)[22]O.Tchernyshyov,R.Moessner and S.L.Sondhi.Phys.Rev.B66,064403(2002).[23]O.Ofer,A.Keren and C.Baines.J.Phys.:Condens.Matter19145270(2007).[24]S.-W.Han,J.S.Gardner,and C.H.Booth.Phys.Rev.B69,024416(2004)[25]J.S.Gardner,B.D.Gaulin and D.McK Paul.J.Crys.Growth191,740(1998).[26]Malcolm F.Collins,Magnetic Critical Scattering,Oxford University Press,New York.(1989)[27]G.A.Gehring and K.A.Gehring,Rep.Prog.Phys.,38,1(1975).[28]Y.Hirano,N.Wakabayashi,C.K.Loong,and L.A.Boat-ner,Phys.Rev.B,67,014423(2003).[29]Michael J.Lewis,Masters Thesis,McMaster University.(2002)。
