详细FLUENT实例讲座-翼型计算
FLUENT翼型算例 - Flow over an Airfoil- Problem Specification教程
FLUENT - Flow over an Airfoil- Problem SpecificationConsider air flowing over NACA 4412 airfoil. The free stream velocity is 50 m/s and the angle of attack is 2°. Assume standard sea-level values for the free stream properties:Pressure = 101,325 PaDensity = 1.2250 kg/m3Temperature = 288.16 KKinematic viscosity v = 1.4607e-5 m2/sWe will determine the lift and drag coefficients under these conditions using FLUENT.Higher Resolution ImageThis tutorial leads you through the steps for generating a mesh in GAMBIT for an airfoil geometry. This mesh can then be read into FLUENT for fluid flow simulation.In an external flow such as that over an airfoil, we have to define a far field boundary and mesh the region between the airfoil geometry and the far field boundary. It is a good idea to place the far field boundary well away from the airfoil since we'll use the ambient conditions to define the boundary conditions at the far field. The farther we are from the airfoil, the less effect it has on the flow and so more accurate is the far field boundary condition.The far field boundary we'll use is the line ABCDEFA in the figure above. c is the chord length.Start GAMBITCreate a new directory called airfoil and start GAMBIT from that directory by typing gambit -id airfoil at the command prompt.Under Main Menu, select Solver > FLUENT 5/6 since the mesh to be created is to be used in FLUENT 6.0.Import EdgeTo specify the airfoil geometry, we'll import a file containing a list of vertices along the surface and have GAMBIT join these vertices to create two edges, corresponding to the upper and lower surfaces of the airfoil. We'll then split these edges into 4 distinct edges to help us control the mesh size at the surface.The file containing the vertices for the airfoil can be downloaded here: naca4412.dat (right click and select Save As...)Let's take a look at the naca4412.dat file:255 20 0 0-0.00019628 0.001241474 0-0.000247836 0.001759397 0-0.000275577 0.002158277 0-0.000290986 0.002495536 0-0.000298515 0.002793415 0-0.000300443 0.00306332 0The first line of the file represents the number of points on each edge (255) and the number of edges (2). The first 255 set of vertices are connected to form the edge corresponding to the upper surface; the next 255 are connected to form the edge for the lower surface.The chord length, c for the geometry in naca4412.dat file is 1, so x varies between 0 and 1. If you are using a different airfoil geometry specification file, note the range of x values in the file and determine the chord length c. You will need this later on.Main Menu > File > Import > ICEM Input ...For File Name, browse and select the naca4412.dat file. Select both Vertices and Edges under Geometry to Create: since these are the geometric entities we need to create. Deselect Face. Click Accept.Higher Resolution ImageWe have more points around the nose area because of the high curvature around the nose.Create Far field BoundaryNext, we will create the following far field boundary. This picture of thefarfield nomenclaturewill be handy.We will create the far field boundary by creating vertices and joining them appropriately to form edges.Operation Tool pad > Geometry Command Button> Vertex Command Button> Create VertexCreate the following vertices by entering the coordinates under Global and the label under Label:Click the FIT TO WINDOW button to scale the display so that you can see all the vertices. The resulting image should look like this:Higher Resolution ImageNow we can create the edges using the vertices created.Operation Tool pad > Geometry Command Button> Edge Command Button> Create EdgeCreate the edge AB by selecting the vertex A followed by vertex B. Enter AB for Label. Click Apply. GAMBIT will create the edge. You will see a message saying something like "Created edge: AB'' in the Transcript window. Similarly, create the edges BC, CD, DE, EG, GA and CG. Note that you might have to zoom in on the airfoil to select vertex G correctly or click on the to select the vertices from the list and move them to the picked list. The rest of the tutorial will use this method for vertices selection.Next we'll create the circular arc AF. Right-click on the Create Edge button and select Arc.In the Create Real Circular Arc menu, the box next to Center will be yellow. That means that the vertex you select will be taken as the center of the arc. Select vertex G and click Apply. Now the box next to End Points will be highlighted in yellow. This means that you can now select the two vertices that form the end points of the arc. Select vertex A and then vertex F. Enter AF under Label. Click Apply.If you did this right, the arc AF will be created. If you look in the transcript window, you'll see a message saying that an edge has been created. Similarly, create an edge corresponding to arc EF.Higher Resolution ImageCreate FacesThe edges we have created can be joined together to form faces. We will need to define three faces as shown in the image above. Two rectangular faces, rect1 and rect2 lie to the right of the airfoil. The third face, circ1 consists of the area outside of the airfoil but inside of the semi-circular boundary.Operation Tool pad > Geometry Command Button> Face Command Button> Form FaceThis brings up the Create Face From Wireframe menu. Recall that we had selected vertices in order to create edges. Similarly, we will select edges in order to form a face.To create the face rect1, select the edges AB, BC, CG, and GA. Enter rect1for the label and click Apply. GAMBIT will tell you that it has "Created face: rect1'' in the transcript window.Similarly, create the face rect2 by selecting ED, DC, CG and GE.To create the last face we will need to make two separate faces, one for the outer boundary and one for the airfoil and then subtract the airfoil from the boundary. Create semi-circular face circ1 by selecting GA, AF, FE and EG and enter circ1 for the label. Create the face for the airfoil by selecting corresponding edges. Subtract the airfoil from circ1.Operation Tool pad > Geometry Command Button> Face Command Buttonright click on the Boolean Operations Button and select SubtractThe Face box will be highlighted yellow. Shift click to select circ1, the outer semi-circular boundary. Then select the lower box labeled Subtract Faces which will allow you to select faces to subtract from our outer boundary. Select the airfoil face and click apply.Mesh FacesWe'll mesh each of the 3 faces separately to get our final mesh. Before we mesh a face, we need to define the point distribution for each of the edges that form the face i.e. we first have to mesh the edges. We'll select the mesh stretching parameters and number of divisions for each edge based on three criteria:1. We'd like to cluster points near the airfoil since this is where the flow is modified the most;the mesh resolution as we approach the far field boundaries can become progressively coarser since the flow gradients approach zero.2. Close to the surface, we need the most resolution near the leading and trailing edgessince these are critical areas with the steepest gradients.3. We want transitions in mesh size to be smooth; large, discontinuous changes in the meshsize significantly decrease the numerical accuracy.The edge mesh parameters we'll use for controlling the stretching are successive ratio, first length and last length. Each edge has a direction as indicated by the arrow in the graphics window. The successive ratio R is the ratio of the length of any two successive divisions in the arrow direction as shown below. Go to the index of the GAMBIT User Guide and look under Edge>Meshing for this figure and accompanying explanation. This help page also explains what the first and last lengths are; make sure you understand what they are.Operation Tool pad > Mesh Command Button> Edge Command Button> Mesh EdgesSelect the edge GA. The edge will change color and an arrow and several circles will appear on the edge. This indicates that you are ready to mesh this edge. Make sure the arrow is pointing upwards. You can reverse the direction of the edge by clicking on the Reverse button in the Mesh Edges menu.Enter a ratio of 1.15. This means that each successive mesh division will be1.15 times bigger in the direction of the arrow. Select Interval Count underSpacing. Enter 45 for Interval Count. Click Apply. GAMBIT will create 45 intervals on this edge with a successive ratio of 1.15.For edges AB and CG, we'll set the First Length (i.e. the length of the division at the start of the edge) rather than the Successive Ratio. Repeat the same steps for edges BC, AB and CG with the following specifications:Note that later we'll select the length at the trailing edge to be 0.02c so that the mesh length is continuous between IG and CG, and HG and CG.Now that the appropriate edge meshes have been specified, mesh the face rect1:Operation Tool pad > Mesh Command Button> Face Command Button> Mesh FacesSelect the face rect1. The face will change color. You can use the defaults of Quad (i.e. quadrilaterals) and Map. Click Apply.The meshed face should look as follows:Higher Resolution ImageNext mesh face rect2 in a similar fashion. The following table shows the parameters to use for the different edges:The resultant mesh should be symmetric about CG as shown in the figure below.Higher Resolution ImageSplit EdgesNext, we will split the top and bottom edges of the airfoil into two edges so that we have better control of the mesh point distribution. Figure of the splitting edges is shown below.We need to do this because a non-uniform grid spacing will be used for x<0.3c and a uniform grid spacing for x>0.3c. To split the top edge into HI and IG, selectOperation Tool pad > Geometry Command Button> Edge Command Button> Split/Merge EdgeMake sure Point is selected next to Split With in the Split Edge window. Select the top edge of the airfoil by Shift-clicking on it.We'll use the point at x=0.3c on the upper surface to split this edge into HI and IG. To do this, enter 0.3 for x: under Global. If your c is not equal to one, enter the value of 0.3*c instead of just 0.3.For instance, if c=4, enter 1.2. From here on, whenever you're asked to enter (some factor)*c, calculate the appropriate value for your c and enter it.Click Apply. You will see a message saying "Edge edge.1 was split, and edge edge.3 created'' in the Transcript window.Repeat this procedure for the lower surface to split it into HJ and JG. Use the point at x=0.3c on the lower surface to split this edge.Higher Resolution ImageFinally, let's mesh the face consisting of circ1 and the airfoil surface. For edges HI and HJ on the front part of the airfoil surface, use the following parameters to create edge meshes:For edges IG and JG, we'll set the divisions to be uniform and equal to 0.02c. Use Interval Size rather than Interval Count and create the edge meshes:For edge AF, the number of divisions needs to be equal to the number of divisions on the line opposite to it, in this case, the upper surface of the airfoil(this is a subtle point; chew over it). To determine the number of divisions that GAMBIT has created on edge IG, selectOperation Tool pad > Mesh Command Button> Edge Command Button>Summarize Edge MeshSelect edge IG and then Elements under Component and click Apply. This will give the total number of nodes (i.e. points) and elements (i.e. divisions) on the edge in the Transcript window. The number of divisions on edge IG is35. (If you are using a different geometry, this number will be different; I'll refer to it as NIG). So the Interval Count for edge AF is NHI+NIG= 40+35= 75. Similarly, determine the number of divisions on edge JG. This comes out as 35 for the current geometry. So the Interval Count for edge EF is 75.Create the mesh for edges AF and EF with the following parameters:Mesh the face. The resultant mesh is shown below.Higher Resolution ImageWe'll label the boundary AFE as farfield1, ABDE as farfield2 and the airfoil surface as airfoil. Recall that these will be the names that show up under boundary zones when the mesh is read into FLUENT.Group EdgesWe'll create groups of edges and then create boundary entities from these groups.First, we will group AF and EF together.Operation Tool pad > Geometry Command Button > Group Command Button > Create GroupSelect Edges and enter farfield1 for Label, which is the name of the group. Select the edges AF and EF.Note that GAMBIT adds the edge to the list as it is selected in the GUI.Click Apply.In the transcript window, you will see the message "Created group: farfield1 group".Similarly, create the other two far field groups. You should have created a total of three groups:Define Boundary TypesNow that we have grouped each of the edges into the desired groups, we can assign appropriate boundary types to these groups.Operation Tool pad > Zones Command Button> Specify Boundary TypesUnder Entity, select Groups.Select any edge belonging to the airfoil surface and that will select the airfoil group. Next to Name:, enter airfoil. Leave the Type as WALL.Click Apply.In the Transcript Window, you will see a message saying "Created Boundary entity: airfoil".Similarly, create boundary entities corresponding to farfield1, farfield2 and farfield3groups. Set Type to Velocity-Inlet for farfield1and farfield2. Set Type to Pressure-Outlet for farfield3.Save Your WorkMain Menu > File > SaveExport MeshMain Menu > File > Export > Mesh...Save the file as airfoil.msh.Make sure that the Export 2d Mesh option is selected.Check to make sure that the file is created.Launch FLUENTStart > Programs > Fluent Inc > FLUENT 6.3.26Select 2ddp from the list of options and click Run.Import FileMain Menu > File > Read > Case...Navigate to your working directory and select the airfoil.msh file. Click OK. The following should appear in the FLUENT window:Check that the displayed information is consistent with our expectations of the airfoil grid.Analyze GridGrid > Info > SizeHow many cells and nodes does the grid have?Display > GridNote what the surfaces farfield1, farfield2, etc. correspond to by selecting and plotting them in turn.Zoom into the airfoil.Where are the nodes clustered? Why?Define PropertiesDefine > Models > Solver...Under the Solver box, select Pressure Based.Click OK.Define > Models > ViscousSelect Inviscid under Model.Click OK.Define > Models > EnergyThe speed of sound under SSL conditions is 340 m/s so that our free stream Mach number is around 0.15. This is low enough that we'll assume that the flow is incompressible. So the energy equation can be turned off.Make sure there is no check in the box next to Energy Equation and click OK.Define > MaterialsMake sure air is selected under Fluid Materials. Set Density to constant and equal to 1.225 kg/m3.Click Change/Create.Define > Operating ConditionsWe'll work in terms of gauge pressures in this example. So set Operating Pressure to the ambient value of 101,325 Pa.Click OK.Define > Boundary ConditionsSet farfield1 and farfield2 to the velocity-inlet boundary type.For each, click Set.... Then, choose Components under Velocity Specification Method and set the x- and y-components to that for the free stream. For instance, the x-component is 50*cos(1.2)=49.99. (Note that 1.2° is used as our angle of attack instead of 2°to adjust for the error caused by assuming the airfoil to be 2D instead of 3D.)Click OK.Set farfield3to pressure-outlet boundary type, click Set...and set the Gauge Pressure at this boundary to 0. Click OK.Solve > Control > SolutionTake a look at the options available.Under Discretization, set Pressure to PRESTO!and Momentum to Second-Order Upwind.(click picture for larger image)Click OK.Solve > Initialize > Initialize...As you may recall from the previous tutorials, this is where we set the initial guess values (the base case) for the iterative solution. Once again, we'll set these values to be equal to those at the inlet (to review why we did this look back to the tutorial about CFG programs). Select farfield1 under Compute From.Click Init.Solve > Monitors > Residual...Now we will set the residual values (the criteria for a good enough solution). Once again, we'll set this value to 1e-06.(click picture for larger image)Click OK.Solve > Monitors > Force...Under Coefficient, choose Lift. Under Options, select Print and Plot. Then, Choose airfoil under Wall Zones.Lastly, set the Force Vector components for the lift. The lift is the force perpendicular to the direction of the free stream. So to get the lift coefficient, set X to -sin(1.2°)=-020942 and Y to cos(1.2°)=0.9998.(click picture for larger image)Click Apply for these changes to take effect.Similarly, set the Force Monitor options for the Drag force. The drag is defined as the force component in the direction of the free stream. So under Force Vector, set X to cos(1.2°)=0.9998 and Y to sin(1.2°)=0.020942 Turn on only Print for it.Report > Reference ValuesNow, set the reference values to set the base cases for our iteration. Select farfield1 under Compute From.Click OK.Note that the reference pressure is zero, indicating that we are measuring gage pressure.Main Menu > File > Write > Case...Save the case file before you start the iterations.Solve > IterateMake note of your findings, make sure you include data such as;What does the convergence plot look like?How many iterations does it take to converge?How does the Lift coefficient compared with the experimental data?Main Menu > File > Write > Case & Data...Save case and data after you have obtained a converged solution.Lift CoefficientThe solution converged after about 480 iterations.476 1.0131e-06 4.3049e-09 1.5504e-09 6.4674e-01 2.4911e-03 0:00:48 524! 477 solution is converged477 9.9334e-07 4.2226e-09 1.5039e-09 6.4674e-01 2.4910e-03 0:00:38 523From FLUENT main window, we see that the lift coefficient is 0.647. This compare fairly well with the literature result of 0.6 from Abbott et al.Plot Velocity VectorsLet's see the velocity vectors along the airfoil.Display > VectorsEnter 4 next to Scale. Enter 3 next to Skip. Click Display.Higher Resolution ImageAs can be seen, the velocity of the upper surface is faster than the velocity on the lower surface.White Background on Graphics WindowTo get white background go to:Main Menu > File > HardcopyMakeselectprompted "Higher Resolution ImageOn the leading edge, we see a stagnation point where the velocity of the flow is nearly zero. The fluid accelerates on the upper surface as can be seen from the change in colors of the vectors.Higher Resolution ImageOn the trailing edge, the flow on the upper surface decelerates and converge with the flow on the lower surface.Do note that the time for fluid to travel top and bottom surface of the airfoil is not necessarily the same, as common misconceptionPlot Pressure CoefficientPressure Coefficient is a dimensionless parameter defined by the equationwhere p is the static pressure,P ref is the reference pressure, andq ref is the reference dynamic pressure defined byThe reference pressure, density, and velocity are defined in the Reference Values panel in Step 5. Please refer to FLUENT's help for more information. Go to Help > User's Guide Index for help.Plot > XY Plot...Change the Y Axis Function to Pressure..., followed by Pressure Coefficient. Then, select airfoil under Surfaces.Click Plot.Higher Resolution ImageThe lower curve is the upper surface of the airfoil and have a negative pressure coefficient as the pressure is lower than the reference pressure.Plot Pressure ContoursPlot static pressure contours.Display > Contours...Select Pressure... and Pressure Coefficient from under Contours Of. Check the Filled and Draw Grid under Options menu. Set Levels to 50.Click Display.Higher Resolution ImageFrom the contour of pressure coefficient, we see that there is a region of high pressure at the leading edge (stagnation point) and region of low pressure on the upper surface of airfoil. This is of what we expected from analysis of velocity vector plot. From Bernoulli equation, we know that whenever there is high velocity, we have low pressure and vise versa.Force ConventionsFLUENT report forces in term of pressure force and viscous force. For instance, we are interested in the drag on the airfoil,(Drag)total = (Drag)pressure + (Drag)viscousDrag due to pressure:Drag due to viscous effect:wheree d is the unit vector parallel to the flow direction.n is unit vector perpendicular to the surface of airfoil.t is unit vector parallel to the surface of airfoil.Similarly, if we are interested in the lift on the airfoil,(Lift) = (Lift)pressure + (Lift)viscousLift due to pressure:Lift due to viscous effect:Report ForceWe will first investigate the Drag on the airfoil.Main Menu > Report > Forces...Select Forces. Under Force Vector, enter 0.9998 next to X. Enter 0.02094 next to Y. Select airfoil under Wall Zones. Click Print.Here's is what we see in the main menu:C d = (C d)pressure + (C d)skin frictionwhere(C d)pressure is due to pressure force.(C d)skin friction is due to viscous force.Indeed, we see that the (C d)skin friction is zero because of the inviscid model.In reality, (Cof the inviscid model that we specify. (Czerocomputation.Now, let's look at the lift coefficient.Main Menu > Report > Forces...Select Forces. Under Force Vector, enter -0.02094 next to X. Enter 0.9998 next to Y. Select airfoil under Wall Zones. Click Print.Here's is what we see in the main menu:Similarly, lift force is due to the contribution of pressure force and viscous force.C l = (C l)pressure + (C l)skin frictionwhere(C l)pressure is due to pressure force.(C l)skin friction is due to viscous force.Since our model is inviscid, (C l)skin friction is zero. We see that the lift coefficient compare well with the experimental value of 0.6.Doexperimental value. In reality, if we take into account the effect of viscosity,Grid ConvergenceA finer mesh with four times the original mesh density was created. The lift coefficient was found to be 0.649.We see that the difference in drag coefficient is very large. We used inviscid case for our model, so we are expecting a C d of zero. However, since the parameter of interest is the lift coefficient, and the value lift coefficient does not deviate much from original mesh to fine mesh, we concluded that the fine mesh is good enough.Thevalidation steps are needed before we can conclude about the accuracy of our model. Other parameter that will affect the validity of our result is the choice of viscous model. We used inviscid model which basically assumed that the flow inviscid and totally ignore the effect of boundary layer near the airfoilReynolds number flow.SummaryFollowing table shows comparison of modeling result with experimental data.Though further validation steps are still needed before we can come up with a model that will accurately represent the physical flow, this simple tutorial demonstrates the use of reasonable assumption and approximation in obtaining understanding of physical flow properties around an airfoil. ReferenceThe experimental data is taken from Theory of Wing Sections By Ira Herbert Abbott, Albert Edward Von Doenhoff pg. 488Google scholar linkConsider the incompressible, inviscid airfoil calculation in FLUENT presented in class. Recall that the angle of attack, α, was 5°.Repeat the calculation for the airfoil for α = 0° and α = 10°. Save your calculation for each angle of attack as a different case file.(a) Graph the pressure coefficient (C p) distribution along the airfoil surface at α = 5° and α = 10° in the manner discu ssed in class (i.e., follow the aeronautical convention of letting C p decrease with increasing ordinate (y-axis) values).What change do you see in the C p distribution on the upper and lower surfaces as you increase the angle of attack?Which part of the airfoil surface contributes most to the increase in lift with increasing α?Hint: The area under the C p vs. x curve is approximately equal to C l.(b) Make a table of C l and C d values obtained for α = 0°, 5°, and 10°. Plot C l vs.α for the three values of α. Make a linear least squares fit of this data and obtain the slope. Compare your result to that obtained from inviscid, thinairfoil theory:,where α is in degrees.Repeat the incompressible calculation at α = 5° including viscous effects. Since the Reynolds number is high, we expect the flow to be turbulent. Use the k-ε turbulence model with the enhanced wall treatment option. At the far field boundaries, set turbulence intensity=1% and turbulent length scale=0.01.(a) Graph the pressure coefficient (C p) distribution along the airfoil surface for this calculation and the inviscid calculation done in the previous problem at α = 5°. Comment on any differences you observe.(b) Compare the C l and C d values obtained with the corresponding values from the inviscid calculation. Discuss briefly the similarities and differences between the two results.。
fluent仿真案例

fluent仿真案例Fluent仿真是一种广泛应用于工程领域的计算流体力学(CFD)软件。
它通过对流动、传热和化学反应等物理过程进行数值模拟,可以帮助工程师们更好地理解和优化各种设备和系统的性能。
下面将列举一些使用Fluent仿真的案例,以展示其在不同领域的应用。
1. 汽车空气动力学优化Fluent仿真可以对汽车外形进行流体力学分析,优化车身设计,降低风阻系数,提高车辆的燃油效率和稳定性。
2. 建筑空调系统设计通过Fluent仿真,可以模拟建筑内部空气流动和热传递,优化空调系统的设计和布局,提高室内空气质量,节约能源消耗。
3. 风力发电机翼型设计Fluent仿真可以模拟风力发电机翼型在风中的流动情况,优化翼型的气动性能,提高风力发电机的发电效率。
4. 燃烧室设计Fluent仿真可以模拟燃烧室内的燃烧过程,优化燃烧室的结构和燃料喷射方式,提高燃烧效率和减少污染物排放。
5. 石油钻井流体力学分析Fluent仿真可以模拟油井中流体的流动和压力变化,帮助工程师们优化钻井参数,提高钻井效率和安全性。
6. 医疗器械设计通过Fluent仿真,可以模拟医疗器械与人体组织的相互作用,优化器械的设计和材料选择,提高治疗效果和患者的舒适度。
7. 液压系统优化Fluent仿真可以模拟液压系统中液体的流动和压力变化,优化管路设计和阀门选择,提高液压系统的效率和响应速度。
8. 船舶流体力学分析通过Fluent仿真,可以模拟船舶在水中的流动情况,优化船体设计和推进系统,提高船舶的航行性能和燃油经济性。
9. 食品加工设备设计Fluent仿真可以模拟食品加工设备内部的流动和传热过程,优化设备的设计和操作参数,提高加工效率和产品质量。
10. 太阳能光伏板优化Fluent仿真可以模拟太阳能光伏板在不同光照条件下的温度分布和功率输出,优化光伏板的设计和散热方式,提高太阳能转换效率。
通过以上案例的描述,可以看出Fluent仿真在多个领域的应用广泛而深入。
fluent处理三维机翼数据及用相关软件绘制三维翼型过程

fluent处理三维机翼数据及用相关软件绘制三维翼型过程Fluent大作业三维翼型扰流实验报告---2008011722李凌尧,说明: 因排版原因,文中部分图形较小可拖大,另外对应不同word排版可能稍改变,目的意义研究了凹凸结节的分布规律对平板舵的水动力性能及失速角的影响,为前缘凹凸结节机翼的优化设计奠定了基础。
模型的建立说明:对于截图,左侧为相应设置,右侧为ANSYS显示。
对于标准机翼做法同理,此报告仅以凹凸机翼的做法为例作说明。
2.1点的选择生成NACA0020数据点,file中打开读入data文件。
凹凸舵点线面的生成 2.2输入点坐标,连接相应点生成曲线,如图: 1Fluent大作业再根据曲线建立面2.3生成流域输入点坐标、连接相应点生成曲线,由相应曲线建立面,然后再生成体如图: 2Fluent大作业2.4生成新的part关闭点和线以及体,只留面。
选择part---create part。
关于面选择见下框: 创建名为POINTS的新Part,关闭线和面,选择所有点创建名为CURVES的新Part,关闭点和面,选择所有线保存File---Geometry---Save Geometry As设定速度入口命名为INLET设定出口命名为OUTLET选择面设定速度入口命名为TOP选择面设定速度入口命名为BOTTOM选择面设定壁面命名为WALL1选择面设定壁面命名为WALL2选择面定义机翼表面名称WING1选择面名称WING2选择面名称WING3选择面名称WING4选择面(说明:在后面fluent设置中WALL1,WALL2也设为流出面)块的划分及网格的生成3.1全选流域,生成block如下图所示:3Fluent大作业3.2切block点击叶片上的一点,点击要切的边,共切3次;同理反方向且两次;然后在另一方向切两次,切后结果如下图:3.3挤压block选择对应的边和块挤压,图示为一例挤压情况: 4Fluent大作业对机翼及整个流域相应的地方挤压完成后如图:3.4删除机翼内部的块。
Fluent翼型算例(中)

翼型流场的流动问题提出考虑空气流过给定的翼型:远前方来流为50m/s,攻角为5°,并假设处于海平面(压强101325Pa,密度1.2250kg/m3,温度288.16k,运动粘度1.4607*10‐5m2/s)。
利用FLUENT确定这些条件下的升力和阻力系数第一步:在GAMBIT中绘制网格几何外形本指导将带领你利用GAMBIT生成一个翼型网格,之后可将本网格导入FLUENT中进行流场计算。
在计算外部层流时,例如翼型上的,我们必须定义一个边界,并将边界与翼型之间的区域划分成网格。
将边界与翼型设置的尽量远是有好处的,因为我们将定义边界条件为环境条件,边界设置的越远,边界对流动的影响越弱,边界条件也就满足的越精确。
我们要用到的边界是上图中ABCDEFA所围成的图形,c是翼型的弦长。
打开GAMBIT创建一个名为“翼型”的新文件夹,打开GAMBIT后,选择文件夹“翼型”为工作文件夹。
在主菜单中选择Solver > FLUNENT 5/6,因为所画网格将用FLUENT6.0计算。
输入边界为了指定翼型几何形状,我们输入一组沿着翼型表面的连续顶点坐标,再通过GAMBIT利用这些坐标生成与翼型的上下表面吻合的两条边,然后将上下表面分成4个不同区域来帮助我们控制表面网格的尺寸。
让我们先来看下文件vertices.dat:文件的第一行表示每边的顶点数(61)和边数(2)。
钱61个顶点会连接形成符合翼型上表面的边,后61个顶点会连接形成符合翼型下表面的边。
在vertices.dat中弦长为1,所以X值在0和1之间,如果你用的是另一个翼型文件,注意X的值,在之后的过程中你可能会需要这样○1。
Main Menu > File > Import > ICEM Input…在点Browse选择要导入的vertices.dat文件,在选中Geometry to Create下的Vertices和Edges,这些是我们需要创建的几何实体,最后点Accept。
Fluent关于飞机的算例 【非常详细】
0.0230 0.0221 0.0211 0.0198 0.0185
变换仰角的计算结果
尾翼和机体交线过于复杂
解决办法
机翼与机体分离
解决办法
围机翼时候U-V失败,解决办法
飞机的几何翻转,注意点,并线时候遇到问题 及解决办法
计算区域
虚面的出现及影响
先将所有体围好后做网格的坏处
有的体很难划分结构化网格,需要对几何 体进行改动 一个体的改动会牵动相邻的体,进而波及 更多的体 ,导致返工
绪言
网格的工具,机器配置
gambit, 双奔3◎866mhz,2G内存
数据处理Biblioteka 原始数据文件给出了点座标。 数据的整理和读入,journel文件
做网格的基本思路
gambit做网格的顺序是点联线,线围面, 面围体,然后切分网格。 nurbs方法联线,net surface (U-V)方法围 面,stitch方法围体。
无量纲化
参数:参考面积s=26.15m2 特征长度 cl=8.32m b=3.79m 动压q=0.5*ρ *v2 F升 M滚 C升 C滚 qs q s cl
F阻 C阻 qs F侧 C侧 qs
C俯 C偏
M俯 q s cl M偏 qsb
工况a: 1.535马赫
来流速度1.535马赫 飞机仰角4度
工况a
工况a
升力系数 试验结果 (未修正) 计算结果 (Euler) 0.2179
阻力系数 0.0390
0.2173
0.0211
压力分布和马赫数图
压力分布和马赫数图
变换仰角的计算结果
仰角(单 0 位:度) 升力 系数 阻力 系数 2 4 6 8
fluent处理三维机翼数据及用相关软件绘制三维翼型过程
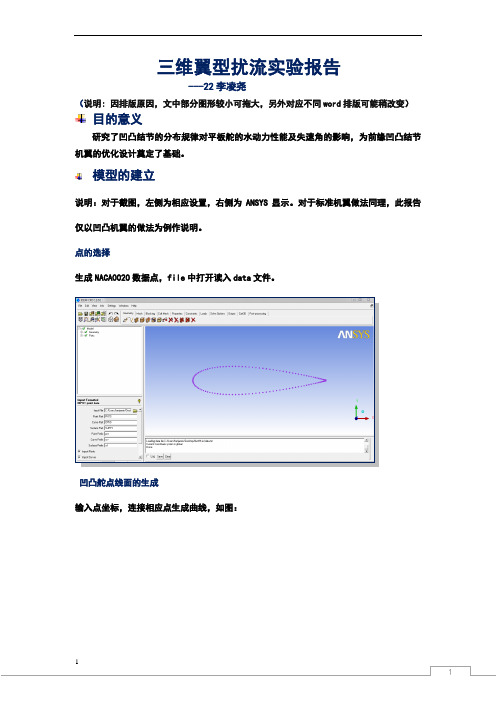
三维翼型扰流实验报告---22李凌尧(说明: 因排版原因,文中部分图形较小可拖大,另外对应不同word排版可能稍改变)目的意义研究了凹凸结节的分布规律对平板舵的水动力性能及失速角的影响,为前缘凹凸结节机翼的优化设计奠定了基础。
模型的建立说明:对于截图,左侧为相应设置,右侧为ANSYS显示。
对于标准机翼做法同理,此报告仅以凹凸机翼的做法为例作说明。
点的选择生成NACA0020数据点,file中打开读入data文件。
凹凸舵点线面的生成输入点坐标,连接相应点生成曲线,如图:再根据曲线建立面生成流域输入点坐标、连接相应点生成曲线,由相应曲线建立面,然后再生成体如图:生成新的part关闭点和线以及体,只留面。
选择part---create part。
关于面选择见下框:创建名为POINTS的新Part,关闭线和面,选择所有点创建名为CURVES的新Part,关闭点和面,选择所有线保存File---Geometry---Save Geometry As设定速度入口命名为INLET设定出口命名为OUTLET选择面设定速度入口命名为TOP选择面设定速度入口命名为BOTTOM选择面设定壁面命名为WALL1选择面设定壁面命名为WALL2选择面定义机翼表面(说明:在后面fluent设置中WALL1,WALL2也设为流出面)块的划分及网格的生成全选流域,生成block如下图所示:切block点击叶片上的一点,点击要切的边,共切3次;同理反方向且两次;然后在另一方向切两次,切后结果如下图:挤压block选择对应的边和块挤压,图示为一例挤压情况:对机翼及整个流域相应的地方挤压完成后如图:删除机翼内部的块。
生成Y型网格(选择Y-block)4和5两步结束后其结果如下图:切边界层选边界层厚度为,可以通过平移机翼上下表面的点来准确得到边界层的厚度。
平移图中所示的点:点确定后,平移,其边界层形成后,整体效果如下图所示:更改边界相对厚度edge A:Parameter=;edge B: Parameter=移动要求的Vertices移动所要求的部分2点使网格质量较高,不出现小于14度的网格。
fluent处理三维机翼数据及用相关软件绘制三维翼型过程
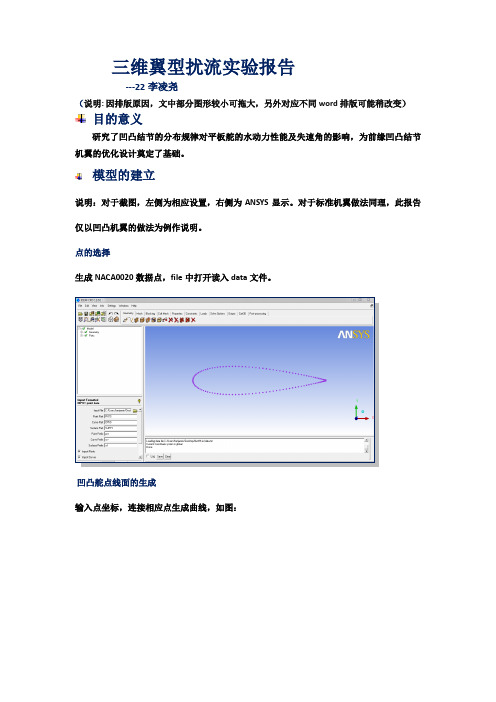
三维翼型扰流实验报告---22李凌尧(说明: 因排版原因,文中部分图形较小可拖大,另外对应不同word排版可能稍改变)目的意义研究了凹凸结节的分布规律对平板舵的水动力性能及失速角的影响,为前缘凹凸结节机翼的优化设计奠定了基础。
模型的建立说明:对于截图,左侧为相应设置,右侧为ANSYS显示。
对于标准机翼做法同理,此报告仅以凹凸机翼的做法为例作说明。
点的选择生成NACA0020数据点,file中打开读入data文件。
凹凸舵点线面的生成输入点坐标,连接相应点生成曲线,如图:再根据曲线建立面生成流域输入点坐标、连接相应点生成曲线,由相应曲线建立面,然后再生成体如图:生成新的part关闭点和线以及体,只留面。
选择part---create part 。
关于面选择见下框: 创建名为POINTS 的新Part ,关闭线和面,选择所有点 创建名为CURVES 的新Part ,关闭点和面,选择所有线 保存File---Geometry---Save Geometry As(说明:在后面fluent 设置中WALL1,WALL2也设为流出面)块的划分及网格的生成设定速度入口命名为INLET设定出口命名为OUTLET 选择面 设定速度入口命名为TOP 选择面设定速度入口命名为BOTTOM 选择面 设定壁面命名为WALL1选择面设定壁面命名为WALL2选择面 定义机翼表面全选流域,生成block如下图所示:切block点击叶片上的一点,点击要切的边,共切3次;同理反方向且两次;然后在另一方向切两次,切后结果如下图:挤压block选择对应的边和块挤压,图示为一例挤压情况:对机翼及整个流域相应的地方挤压完成后如图:删除机翼内部的块。
生成Y型网格(选择Y-block)4和5两步结束后其结果如下图:切边界层选边界层厚度为,可以通过平移机翼上下表面的点来准确得到边界层的厚度。
平移图中所示的点:点确定后,平移,其边界层形成后,整体效果如下图所示:更改边界相对厚度edge A:Parameter=;edge B: Parameter=移动要求的Vertices移动所要求的部分2点使网格质量较高,不出现小于14度的网格。
Fluent翼型算例-Modeling Compressible Flow over an Airfoil教程

Tutorial5.Modeling Compressible Flow over an AirfoilIntroductionThe purpose of this tutorial is to illustrate the setup and solution of an external com-pressibleflow.This tutorial demonstrates how to do the following:•Model compressibleflow.•Set boundary conditions for external aerodynamics.•Use force monitors to judge convergence.•Check the grid by plotting the distribution of Y+.•Adapt the mesh to meet Y+requirements.The study offlow over an airfoil is crucial in the design of airplanes.The correct shape of airfoils has a large impact on the performance of an airplane.There are different flow regimes depending on the free stream conditions.The important parameters in the study of airfoils are the drag and lift forces on the airfoil.Every airfoil performs best ina specific operating range;selection of airfoil depends on the operating conditions. PrerequisitesThis tutorial assumes that you have little experience with FLUENT but are familiar with the interface.Problem DescriptionIn this tutorial,we consider theflow around a NACA2415airfoil(Figures5.1and5.2) at an angle of attack a=10◦and a free stream Mach number(M∞)of0.4066and0.8.The chord length(C)is1m.Modeling Compressible Flow over an AirfoilFigure5.1:Problem SchematicFigure5.2:Airfoil DetailsModeling Compressible Flow over an AirfoilPreparation1.Copy the meshfile,airfoil.msh to your working folder.2.Start the2D double precision(2ddp)version of FLUENT.Setup and SolutionStep1:Grid1.Read the meshfile,airfoil.msh.File−→Read−→Case...FLUENT will read the meshfile and report the progress in the console window.2.Check the grid.Grid−→CheckThis procedure checks the integrity of the mesh.Make sure the reported minimumvolume is a positive number.3.Scale the grid.Grid−→Scale...Check the domain extents to see if they correspond to the actual physical dimensions.Otherwise the grid has to be scaled with proper units.Modeling Compressible Flow over an Airfoil4.Display the grid(Figure5.3).Display−→Grid...(a)Click Display and close the Grid Display panel.Figure5.3:Grid DisplayUse the middle mouse button to zoom in on the image so that the mesh nearairfoil can be viewed.Step2:Models1.Set the solver settings.Define−→Models−→Solver...(a)Click OK to retain the default settings.Modeling Compressible Flow over an Airfoil2.Enable the Spalart-Allmaras turbulence model.Define−→Models−→Viscous...(a)Enable Spalart-Allmaras(1eqn)in the Model list.(b)Click OK to close the Viscous Model panel.The Spalart-Allamaras model is a simple one-equation model that solves amodeled transport equation for the kinematic eddy(turbulent)viscosity.TheSpalart-Allmaras model was designed for aerospace applications involving wall-boundedflows and has shown to give good results for boundary layers subjectedto adverse pressure gradients.Step3:MaterialsThe default workingfluid material in this problem is air.The default settings need to be modified to account for compressibility and variations of the thermophysical properties with temperature.Define−→Materials...Modeling Compressible Flow over an Airfoil1.Select ideal-gas from the Density drop-down list.2.Select sutherland from the Viscosity drop-down list.(a)Click OK to accept the default Three Coefficient Method and other parameters.3.Click Change/Create and close the Materials panel.Density and Viscosity have been made temperature-dependent.For high-speed com-pressibleflows,thermal dependency of the physical properties is recommended.Butfor simplicity,Thermal Conductivity and Cp are assumed to be constant in this case.Step4:Operating ConditionsDefine−→Operating Conditions...Modeling Compressible Flow over an Airfoil1.Set the Operating Pressure to0pascal.Note:For compressibleflows it is recommended to set the operating pressure to zero,to minimize the errors due to pressurefluctuations.Step5:Boundary ConditionsDefine−→Boundary Conditions...1.Set the boundary condition for pressure-far-field(far-field).(a)Select far-field from the Zone list.This Type will be reported as pressure-far-field.(b)Click the Set...button.Modeling Compressible Flow over an AirfoilThe flow Reynolds number is 1e+5and the velocity can be calculated using the expression:Re =ρUH µi.Enter 101325pascal for the Gauge Pressure .ii.Enter 0.4066for the Mach Number .iii.Enter 0.984807for X-component of Flow Direction and 0.173648for Y-component of Flow Direction .As the angle of attack is 10◦,X component of flow direction =1.0×cos (10◦)and Y component of flow direction =1.0×sin (10◦)iv.Select Turbulent Viscosity Ratio from the Specification Method drop-downlist.v.Retain the default value of 10for Turbulent Viscosity Ratio .For external flows,Turbulent Viscosity Ratio should be set between 1and 10.vi.Click OK to close the Pressure Far Field panel.2.Close the Boundary Conditions panel.Modeling Compressible Flow over an AirfoilStep6:Solution1.Retain default solver settings for initial solution...Solve−→Controls−→Solution...2.Initialize theflow.Solve−→Initialize−→Initialize...(a)Select far-field from the Compute From drop-down list.For airfoil simulations,initialize theflowfield using the pressure-far-field bound-ary as it helps in faster convergence.(b)Click Init and close the Solution Initialization panel.3.Enable the plotting of residuals during the calculation.Solve−→Monitors−→Residual...Modeling Compressible Flow over an Airfoil(a)Enable Plot from the Options list.(b)Click OK to close the Residual Monitors panel.4.Save the casefile(airfoil1.cas.gz).File−→Write−→Case...Retain the default Write Binary Files option so that you can write a binaryfile.The.gz extension will save compressedfiles on both,Windows and UNIX platforms.5.Start the calculation by requesting250iterations.Solve−→Iterate...(a)Set the Number of Iterations to250.(b)Click Iterate.(c)Close the Iterate panel after the completion of iterations.Iterate till the solution converges for default convergence criteria.The solutionwill converge in about176iterations.The residuals plot is shown in Figure5.4.Figure5.4:Scaled Residuals6.Set the reference values used to compute the lift,drag and moment coefficients.The reference values are used to non-dimensionalize the forces and moments acting on the airfoil.Report−→Reference Values...(a)Select far-field from the Compute From drop-down list.FLUENT will update the Reference Values based on the boundary conditions at the far-field boundary.(b)Click OK to close the Reference Values panel.7.Check the lift coefficient.Report−→Forces...(a)Select airfoil-bottom and airfoil-top from the Wall Zones list.(b)Enter0.173648for X and0.984807for Y in the Force Vector list.This is because the lift force is in the direction normal to theflow.For the flow incident at10◦,the normal force components will be X=1.0×sin(10◦) and Y=1.0×cos(10◦).(c)Click Print.FLUENT prints the force components and coefficient components in the console window.In the extreme right total coefficient is displayed.It reports a value of0.9726.The expected lift coefficient for NACA-2415for current operating conditions is about1.25[1].8.Set the solution controls.Solve−→Controls−→Solution...(a)Select Second Order Upwind from the Density,Momentum,Modified TurbulentViscosity and Energy drop-down lists.(b)Click OK to close the Solution Controls panel.To predict the correct drag and lift coefficients,it is important to solve with higher order schemes for key parameters of interest.However,from stability perspective,density was selected to start with.9.Specify Convergence Criteria for all the equations.Solve−→Monitors−→Residual...(a)Deselect Check Convergence for all the equations.(b)Click OK to close the Residual Monitors panel.10.Set the monitors for lift and drag coefficients.Solve−→Monitors−→Force...(a)Select airfoil-bottom and airfoil-top from the Wall Zones list.(b)Enter0.984807for X and0.173648for Y in the Force Vector group box.These magnitudes ensure that the lift and drag coefficients are calculated nor-mal and parallel respectively to theflow.(c)Select Drag from the Coefficient drop-down list.(d)Enable Plot and Write in the Options list.(e)Set Plot Window to1.(f)Enter cd-history for the File Name.(g)Click Apply.(h)Similarly,set the monitor for Lift.Note:For Lift,under Force Vector,use the values0.173648and0.984807 for X and Y respectively and set Plot Window to2.Thefilename iscl-history.11.Iterate the solution till the drag and lift coefficients have reached a constant valueas shown in Figures5.5and5.6.This is observed at about1400iterations.12.Check the Y+values around airfoil(Figure5.7).Plot−→XY...Figure5.5:Lift Coefficient PlotFigure5.6:Drag Coefficient Plot(a)Select Turbulence...and Wall Yplus from the Y Axis Function lists.(b)Select airfoil-bottom and airfoil-top in the Surfaces list.(c)Deselect Node Values.Wall Yplus is available only for cell values.(d)Click Plot.Figure5.7:Wall Yplus PlotThe values of Y+are dependent on the grid and the Reynolds number of the flow,and are meaningful only in boundary layers.The value of Y+in the wall-adjacent cells dictates how wall shear stress is calculated.For Spalart-Allmaras,Y+should either be very small(of the order of Y+=1)or greater than30.In this case,the Y+values are slightly high and maximum is about250.Thelift coefficient can be better predicted if the Y+value is kept in the range of30-150.In order to meet this condition,refine the cells adjacent to wall usingAdapt.13.Save the case and datafiles(airfoil2.cas.gz and airfoil2.dat.gz).File−→Write−→Case&Data...14.Adapt a single layer of cells adjacent to airfoil.Adapt−→Boundary...(a)Select airfoil-bottom and airfoil-top in the Boundary Zones list.(b)Click Mark.(c)Click Adapt.(d)Click Yes in the Question dialog box that opens.(e)Close the Boundary Adaption panel.FLUENT will break each cell into four parts such that thefirst cell is at halfthe distance as compared to the original mesh,as shown in Figure5.8.It canbe seen that a non-conformality exists in the mesh after adaption.15.Iterate the solution till the monitors for lift and drag coefficients reach a constantvalue.The coefficients reach a constant value at about3000iterations.Thefinal lift coefficient obtained was about1.146.16.Save the case and datafiles(airfoil3.cas.gz and airfoil3.dat.gz).File−→Write−→Case&Data...Figure5.8:Adapted GridStep7:Postprocessing1.Check the Y+values(Figure5.9).Plot−→XY Plot...(a)Select Turbulence...and Wall Yplus in the Y Axis Function lists.(b)Select airfoil-bottom and airfoil-top in the Surfaces list.(c)Deselect Node Values.(d)Click Plot and close the Solution XY Plot panel.Figure5.9:Wall Yplus Plot After AdaptionAfter adaption,the Y+values have reduced and most of the cells are below130.This shows that the grid resolution is well within the recommended range.2.Display velocity vectors(Figure5.10).Display−→Vectors...(a)Click Vector Options....i.Select Fixed Length.Set the Fixed Length option to display all the vectors with the same length.ii.Click Apply and close the Vector Options panel.(b)Enter0.04for the Scale and2for Skip.Increase Skip to reduce the number of vectors,so that the display does not appear cluttered due to large number of vectors.(c)Click Display and close the Vectors panel.Figure5.10:Velocity Vectors3.Display pressure distribution(Figure5.11).Display−→Contours...(a)Select Pressure...and Static Pressure from the Contours of drop-down lists.(b)Enable Filled in the Options list.(c)Click Display.An additional simulation was performed with free stream Mach number of0.8 at same angle of attack(10).The Mach Number Contour and Velocity Vector for this simulation are shown in Figures5.12and5.13respectively.Figure5.11:Contours of Static PressureThe upper surface of airfoil clearly shows the shock andflow reversal.Shock reduces the lift coefficient on the airfoil due to theflow reversal and hence is undesirable(Figure5.13). The lift coefficient with these conditions was0.7638.Figure5.12:Contours of Mach NumberFigure5.13:Velocity VectorsSummaryTheflowfield at airfoil is highly dependent on the free stream condition.In order to predict correct lift and drag coefficients higher order discretization schemes should be used.On the mesh part it is important to resolve the near wall cell,such that Y+is about1.Due to the mesh count constraint of30,000cells,it is not possible to resolve the mesh further.With the current mesh,results are deviating about8%from the expected values,butflow features are captured correctly.References[1]J.D.Anderson,Jr.,Introduction to Flight,McGraw Hill Higher Education. Exercises/Discussions1.What will be the lift and drag coefficients for different angles of attack.Runthe simulations with a range of angle of attack to trace the complete curve andpredict the stall angle for NACA-2415.Verify its match with the values reportedin literature.2.Simulate theflow with different turbulence models and compare their performancefor drag and lift predictions.3.Run the simulation with different Mach numbers at free stream.4.Coupled solver can be used for higher Mach numberflows and the results can thenbe compared with segregated solver.5.Check for unsteadinessflow features when angle of attack is increased.Links for Further Reading•http://airtraffi:8080/airfoil.htm。
基于Fluent对无人机翼型的计算方法研究

图 2 G ig n生 成 的翼 型 网格 rd e
实现。如果我们选择更合适 的模型并布置合理的计算 网格, 我们就有 可能最大限度地利用我们现有的资源,得到最为可 靠的计算 结果 ,如何能够达 到最佳效果 ,暂时还有没有更好
通过离散的方法 来求解上述方程 ,本文中对 于对 流项采 用的是二阶迎风格式,其具体 的计算过程如下所示 :
U>0
攻角 ( ) 度
9。 7。
升 力系 数 ( 1 C)
l 57 e 0 1 9 + 0 9 7 33 一 . 4 e 0i
阻力 系数 (d C)
5 22 2 一 . 4 e 03 3 23 3 一 . 4 e 03
l 时,连续方程参 数和升力阻力系数不能继续收敛所 以计 0度
算取值点受到一定的局限,出现 失速 现象,计算值 与试验参 数相差较大,在未失速时相对与实验数据结果基本符合,升 力随阻力 的变化趋势也比较接近于实验所得的极 曲线 。
( )结论 五
综上所述 ,F un 可以计算未失速情况下的所有翼型数 le t
2. 6 9 O 1 2 e— 3 3. 9 7 0 0 2 e- 3
—
U < O
图 1 迎 风 格 式 示 意 图
根据 以上表格数据得到翼形的升力系数随攻角变化 曲线 图和极 曲线。如 图 3 4 和 。另外可以直接 由 f u n 软件得到 let 压力沿着机翼表面 的压力系数分布 图;这里只给出 5度时的 压力分布情况 ,从图中可 以看 出 l 时翼型压力分布开始发 0度 散 ,证明在这个角度的时候开始失速 。
fluent处理三维机翼数据及用相关软件绘制三维翼型过程
三维翼型扰流实验报告---2008011722李凌尧(说明: 因排版原因,文中部分图形较小可拖大,另外对应不同word排版可能稍改变)目的意义研究了凹凸结节的分布规律对平板舵的水动力性能及失速角的影响,为前缘凹凸结节机翼的优化设计奠定了基础。
模型的建立说明:对于截图,左侧为相应设置,右侧为ANSYS显示。
对于标准机翼做法同理,此报告仅以凹凸机翼的做法为例作说明。
2.1点的选择生成NACA0020数据点,file中打开读入data文件。
2.2 凹凸舵点线面的生成输入点坐标,连接相应点生成曲线,如图:再根据曲线建立面2.3生成流域输入点坐标、连接相应点生成曲线,由相应曲线建立面,然后再生成体如图:2.4生成新的part关闭点和线以及体,只留面。
选择part---create part。
关于面选择见下框:创建名为POINTS的新Part,关闭线和面,选择所有点创建名为CURVES的新Part,关闭点和面,选择所有线保存File---Geometry---Save Geometry As(说明:在后面fluent 设置中W ALL1,W ALL2也设为流出面) 块的划分及网格的生成3.1全选流域,生成block 如下图所示:3.2切block点击叶片上的一点,点击要切的边,共切3次;同理反方向且两次;然后在另一方向切两次,切后结果如下图:设定速度入口命名为INLET设定出口命名为OUTLET 选择面 设定速度入口命名为TOP 选择面设定速度入口命名为BOTTOM 选择面 设定壁面命名为W ALL1选择面设定壁面命名为W ALL2选择面 定义机翼表面名称WING1选择面 名称WING2选择面名称WING3选择面 名称WING4选择面3.3挤压block选择对应的边和块挤压,图示为一例挤压情况:对机翼及整个流域相应的地方挤压完成后如图:3.4删除机翼内部的块。
3.5生成Y型网格(选择Y-block)4和5两步结束后其结果如下图:3.6切边界层选边界层厚度为0.004,可以通过平移机翼上下表面的点来准确得到边界层的厚度。
Fluent翼型算例(中)

翼型流场的流动问题提出考虑空气流过给定的翼型:远前方来流为50m/s,攻角为5°,并假设处于海平面(压强101325Pa,密度1.2250kg/m3,温度288.16k,运动粘度1.4607*10‐5m2/s)。
利用FLUENT确定这些条件下的升力和阻力系数第一步:在GAMBIT中绘制网格几何外形本指导将带领你利用GAMBIT生成一个翼型网格,之后可将本网格导入FLUENT中进行流场计算。
在计算外部层流时,例如翼型上的,我们必须定义一个边界,并将边界与翼型之间的区域划分成网格。
将边界与翼型设置的尽量远是有好处的,因为我们将定义边界条件为环境条件,边界设置的越远,边界对流动的影响越弱,边界条件也就满足的越精确。
我们要用到的边界是上图中ABCDEFA所围成的图形,c是翼型的弦长。
打开GAMBIT创建一个名为“翼型”的新文件夹,打开GAMBIT后,选择文件夹“翼型”为工作文件夹。
在主菜单中选择Solver > FLUNENT 5/6,因为所画网格将用FLUENT6.0计算。
输入边界为了指定翼型几何形状,我们输入一组沿着翼型表面的连续顶点坐标,再通过GAMBIT利用这些坐标生成与翼型的上下表面吻合的两条边,然后将上下表面分成4个不同区域来帮助我们控制表面网格的尺寸。
让我们先来看下文件vertices.dat:文件的第一行表示每边的顶点数(61)和边数(2)。
钱61个顶点会连接形成符合翼型上表面的边,后61个顶点会连接形成符合翼型下表面的边。
在vertices.dat中弦长为1,所以X值在0和1之间,如果你用的是另一个翼型文件,注意X的值,在之后的过程中你可能会需要这样○1。
Main Menu > File > Import > ICEM Input…在点Browse选择要导入的vertices.dat文件,在选中Geometry to Create下的Vertices和Edges,这些是我们需要创建的几何实体,最后点Accept。
【详细FLUENT实例讲座】翼型计算 - FLUENT - CAE联盟论坛_CAE工程师社区
bell0326,您好! 2条
可用分:-5 专家分:3 退出
查看新帖 精华帖区 我的社区 我感兴趣的论坛 HyperWorks 专区 ANSYS专区 LMS专区 通用有限元分析 前后处理
CFD FLUENT CFD前处理 CFD求解
图1计算模型示意图
1.2 FLUENT前处理设置
Step 1:导入计算模型 以3D,双精度方式启动FLUENT14.5。 利用菜单【File】>【Read】>【Mesh…】,在弹出的文件选择对话框中选择网格文件rae2822_coarse.msh,点击OK按 文件。如图2所示。
点击FLUENT模型树按钮General,在右侧设置面板中点击按钮Display…,在弹出的设置对话框中保持默认设置,点 击Display按钮,显示网格。如图3所ls设置 设置气体密度为理想气体类型。
图8湍流设置
如图9所示,点击FLUENT模型树节点Materials,在右侧设置面板中选择材料air,点击按钮Create/Edit…,弹出材料设 话框。如图10所示。 设置密度Density选项为ideal-gas,设置粘性Viscosity选项为sutherland,在弹出的相应面板中采取默认设置。点 击Change/Create按钮完成材料属性的编辑。
振动噪声(NVH) 碰撞冲击 多体机构 材料成型 优化与疲劳可靠性 热与电磁 其它CAE应用 闲聊区 论坛事务 历史版块(只读)
CAE联盟论坛_CAE工程师社区 » CFD » FLUENT » 【详细FLUENT实例讲座】翼型计算
快速搜索 输入搜索关键字
12345678
Fluent用于飞机计算的算例_【非常详细】
尾翼和机体交线过于复杂
解决办法
机翼与机体分离
解决办法
围机翼时候U-V失败,解决办法
飞机的几何翻转,注意点,并线时候遇到问题 及解决办法
计算区域
虚面的出现及影响
先将所有体围好后做网格的坏处
有的体很难划分结构化网格,需要对几何 体进行改动 一个体的改动会牵动相邻的体,进而波及 更多的体 ,导致返工
来流速度1.535马赫 飞机仰角4度
工况a
工况a
升力系数 试验结果 (未修正) 计算结果 (Euler) 0.2179
阻力系数 0.0390
0.2173
0.0211
压力分布和马赫数图
压力分布和马赫数图
变换仰角的计算结果
仰角(单 0 位:度) 升力 系数 阻力 系数 2 4 6 8
0.0305 0.1250 0.2173 0.3138 0.4101
0.0230 0.0221 0.0211 0.0198 0.0185
变换仰角的计算结果
网格先从机翼处开始做的好处
机翼根部网格最为复杂 从两头往里做的话,一旦到这儿遇到问题 难以避开的话,势必要返工前面的网格。
交错网格的问题
交错网格的问题
交错网格的解决办法
网格的完成
网格的简化
边条件
利用Fluent进行计算
理想气体 无粘模式 气体性质(空气): R=286.85 γ =1.4 T=300K P=101325ห้องสมุดไป่ตู้a ρ =1.1774kg/m3 当地音速a0=347.0979688m/s Re=0.27531e+7 估计边界层厚度为0.005m
无量纲化
参数:参考面积s=26.15m2 特征长度 cl=8.32m b=3.79m 动压q=0.5*ρ *v2 F升 M滚 C升 C滚 qs q s cl
详细FLUENT实例讲座-翼型计算
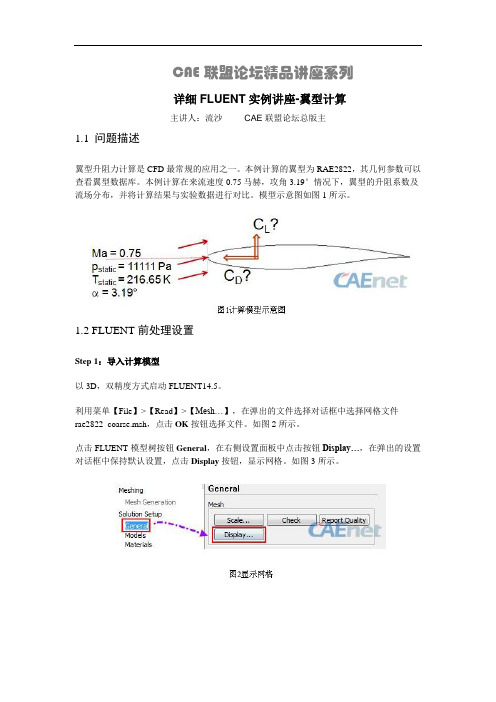
CAE联盟论坛精品讲座系列详细FLUENT实例讲座-翼型计算主讲人:流沙CAE联盟论坛总版主1.1 问题描述翼型升阻力计算是CFD最常规的应用之一。
本例计算的翼型为RAE2822,其几何参数可以查看翼型数据库。
本例计算在来流速度0.75马赫,攻角3.19°情况下,翼型的升阻系数及流场分布,并将计算结果与实验数据进行对比。
模型示意图如图1所示。
1.2 FLUENT前处理设置Step 1:导入计算模型以3D,双精度方式启动FLUENT14.5。
利用菜单【File】>【Read】>【Mesh…】,在弹出的文件选择对话框中选择网格文件rae2822_coarse.msh,点击OK按钮选择文件。
如图2所示。
点击FLUENT模型树按钮General,在右侧设置面板中点击按钮Display…,在弹出的设置对话框中保持默认设置,点击Display按钮,显示网格。
如图3所示。
Step 2:检查网格采用如图4所示步骤进行网格的检查与显示。
点击FLUENT模型树节点General节点,在右侧面板中通过按钮Scale…、Check及Report Quality实现网格检查。
点击按钮Check,在命令输出按钮出现如图5所示网格统计信息。
从图中可以看出,网格尺寸分布:x轴:-48.97~50my轴:0~0.01mz轴:-50~50m符合尺寸要求,无需进行尺寸缩放。
最小网格体积参数minimum volume为1.690412e-9,为大于0的值,符合计算要求。
Step 3:General设置点击模型树节点General,在右侧设置面板中Solver下设置求解器为Density-Based,如图6所示。
小提示:对于高速可压缩流场计算,常常使用密度基求解器。
Step 4:Models设置使用SST k-w湍流模型,并且激活能量方程。
1、激活SST k-w湍流模型如图6-7所示,点击模型树节点Models,在右侧面板中的models列表项中鼠标双击Viscous-laminar,弹出如图6-8所示粘性模型设置对话框,在model选项中选择k-omega(2 eqn),并在k-omega Model选项中选择选项SST,其它参数保持默认。
Fluent软件的使用(3)

空客A320
增升装置形式对升力系数的贡献
CL
后缘襟翼作用
ΔCL
前缘缝翼作用
Δαm
ax
α
增升装置空气动力特性
缝翼尾迹 主翼边界层
主翼尾迹 尾迹与边界层 干扰 逆压梯度中的尾迹发展 可能的激波边界层干扰 流线曲率 襟翼分离
分离泡
转捩 缝隙效应
凹角分离流动 转捩
分离
计算结果
气动力系数:
总的升力、阻力、俯仰力矩 各段翼型的气动力系数比例
总的升力阻力俯仰力矩各段翼型的气动力系数比例翼型表面压力系数分布曲线流场压力和速度云图流场的速度矢量图尤其缝道位置流线图尤其缝道位置
航空工程大型通用软件
计算流体力学FLUENT软件
教 师:郭昊 办公室:新主楼C1114 电 话:82339592 电 邮:guohao@
计算条件
翼型表面压力系数分布曲线 流场压力和速度云图 流场的速度矢量图(尤其缝道位置) 流线图(尤其缝道位置)
提示
Ma数范围比较大 压 PISO Coupled
密度基
30P30N翼型 马赫数Ma=0.2 迎角6度 飞行高度H=0km
α 6
0.05
Y(m)
0.00 -0.05 -0.1 0.0 0.1 0.2 0.3 X(m) 0.4 0.5 0.6
cosα 0.99452
sinα 0.10453
常用民用客机的增升装置
波音747
波音737
空客A380
详细FLUENT实例讲座-翼型详细计算

CAE联盟论坛精品讲座系列详细FLUENT实例讲座-翼型计算主讲人:流沙CAE联盟论坛总版主1.1 问题描述翼型升阻力计算是CFD最常规的应用之一。
本例计算的翼型为RAE2822,其几何参数可以查看翼型数据库。
本例计算在来流速度0.75马赫,攻角3.19°情况下,翼型的升阻系数及流场分布,并将计算结果与实验数据进行对比。
模型示意图如图1所示。
1.2 FLUENT前处理设置Step 1:导入计算模型以3D,双精度方式启动FLUENT14.5。
利用菜单【File】>【Read】>【Mesh…】,在弹出的文件选择对话框中选择网格文件rae2822_coarse.msh,点击OK按钮选择文件。
如图2所示。
点击FLUENT模型树按钮General,在右侧设置面板中点击按钮Display…,在弹出的设置对话框中保持默认设置,点击Display按钮,显示网格。
如图3所示。
Step 2:检查网格采用如图4所示步骤进行网格的检查与显示。
点击FLUENT模型树节点General节点,在右侧面板中通过按钮Scale…、Check及Report Quality实现网格检查。
点击按钮Check,在命令输出按钮出现如图5所示网格统计信息。
从图中可以看出,网格尺寸分布:x轴:-48.97~50my轴:0~0.01mz轴:-50~50m符合尺寸要求,无需进行尺寸缩放。
最小网格体积参数minimum volume为1.690412e-9,为大于0的值,符合计算要求。
Step 3:General设置点击模型树节点General,在右侧设置面板中Solver下设置求解器为Density-Based,如图6所示。
小提示:对于高速可压缩流场计算,常常使用密度基求解器。
Step 4:Models设置使用SST k-w湍流模型,并且激活能量方程。
1、激活SST k-w湍流模型如图6-7所示,点击模型树节点Models,在右侧面板中的models列表项中鼠标双击Viscous-laminar,弹出如图6-8所示粘性模型设置对话框,在model选项中选择k-omega(2 eqn),并在k-omega Model选项中选择选项SST,其它参数保持默认。
Fluent关于飞机的非常详细的算例

无量纲化
参数:参考面积s=26.15m2 特征长度 cl=8.32m b=3.79m 动压q=0.5*ρ *v2 F升 M滚 C升 C滚 qs q s cl
F阻 C阻 qs F侧 C侧 qs
C俯 C偏
M俯 q s cl M偏 qsb
工况a: 1.535马赫
尾翼和机体交线过于复杂尾翼和机体交线过于复杂解决办法解决办法机翼与机体分离机翼与机体分离解决办法解决办法围机翼时候围机翼时候uuvv失败解决办法失败解决办法飞机的几何翻转注意点并线时候遇到问题飞机的几何翻转注意点并线时候遇到问题及解决办法及解决办法计算区域计算区域虚面的出现及影响虚面的出现及影响先将所有体围好后做网格的坏处先将所有体围好后做网格的坏处有的体很难划分结构化网格需要对几何有的体很难划分结构化网格需要对几何体进行改动体进行改动一个体的改动会牵动相邻的体进而波及一个体的改动会牵动相邻的体进而波及更多的体更多的体导致返工导致返工网格先从机翼处开始做的好处网格先从机翼处开始做的好处机翼根部网格最为复杂机翼根部网格最为复杂从两头往里做的话一旦到这儿遇到问题从两头往里做的话一旦到这儿遇到问题难以避开的话势必要返工前面的网格
来流速度1.535马赫 飞机仰角4度
工况a
工况a
升力系数 试验结果 (未修正) 计算结果 (Euler) 0.2179
阻力系数 0.0390
0.217
变换仰角的计算结果
仰角(单 0 位:度) 升力 系数 阻力 系数 2 4 6 8
0.0305 0.1250 0.2173 0.3138 0.4101
绪言
网格的工具,机器配置
gambit, 双奔3◎866mhz,2G内存
数据处理
原始数据文件给出了点座标。 数据的整理和读入,journel文件
fluent处理三维机翼数据及用相关软件绘制三维翼型过程
三维翼型扰流实验报告---22李凌尧(说明: 因排版原因,文中部分图形较小可拖大,另外对应不同word排版可能稍改变)目的意义研究了凹凸结节的分布规律对平板舵的水动力性能及失速角的影响,为前缘凹凸结节机翼的优化设计奠定了基础。
模型的建立说明:对于截图,左侧为相应设置,右侧为ANSYS显示。
对于标准机翼做法同理,此报告仅以凹凸机翼的做法为例作说明。
点的选择生成NACA0020数据点,file中打开读入data文件。
凹凸舵点线面的生成输入点坐标,连接相应点生成曲线,如图:再根据曲线建立面生成流域输入点坐标、连接相应点生成曲线,由相应曲线建立面,然后再生成体如图:生成新的part关闭点和线以及体,只留面。
选择part---create part 。
关于面选择见下框: 创建名为POINTS 的新Part ,关闭线和面,选择所有点 创建名为CURVES 的新Part ,关闭点和面,选择所有线 保存File---Geometry---Save Geometry As(说明:在后面fluent 设置中WALL1,WALL2也设为流出面)块的划分及网格的生成全选流域,生成block 如下图所示:设定速度入口命名为INLET设定出口命名为OUTLET 选择面 设定速度入口命名为TOP 选择面 设定速度入口命名为BOTTOM 选择面设定壁面命名为WALL1选择面 设定壁面命名为WALL2选择面 定义机翼表面名称WING1选择面 名称WING2选择面名称WING3选择面名称WING4选择面切block点击叶片上的一点,点击要切的边,共切3次;同理反方向且两次;然后在另一方向切两次,切后结果如下图:挤压block选择对应的边和块挤压,图示为一例挤压情况:对机翼及整个流域相应的地方挤压完成后如图:删除机翼内部的块。
生成Y型网格(选择Y-block)4和5两步结束后其结果如下图:切边界层选边界层厚度为,可以通过平移机翼上下表面的点来准确得到边界层的厚度。
Fluent翼型算例——超级经典的算例
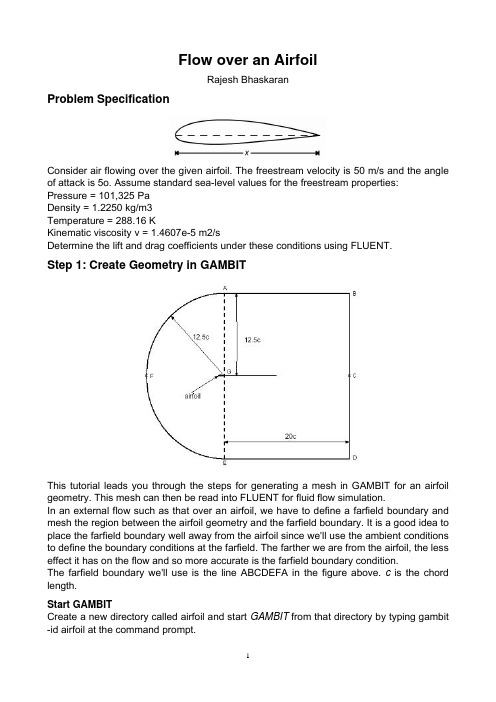
Flow over an AirfoilRajesh BhaskaranProblem SpecificationConsider air flowing over the given airfoil. The freestream velocity is 50 m/s and the angle of attack is 5o. Assume standard sea-level values for the freestream properties:Pressure = 101,325 PaDensity = 1.2250 kg/m3Temperature = 288.16 KKinematic viscosity v = 1.4607e-5 m2/sDetermine the lift and drag coefficients under these conditions using FLUENT.Step 1: Create Geometry in GAMBITThis tutorial leads you through the steps for generating a mesh in GAMBIT for an airfoil geometry. This mesh can then be read into FLUENT for fluid flow simulation.In an external flow such as that over an airfoil, we have to define a farfield boundary and mesh the region between the airfoil geometry and the farfield boundary. It is a good idea to place the farfield boundary well away from the airfoil since we'll use the ambient conditions to define the boundary conditions at the farfield. The farther we are from the airfoil, the less effect it has on the flow and so more accurate is the farfield boundary condition.The farfield boundary we'll use is the line ABCDEFA in the figure above. c is the chord length.Start GAMBITCreate a new directory called airfoil and start GAMBIT from that directory by typing gambit -id airfoil at the command prompt.Under Main Menu, select Solver > FLUENT 5/6 since the mesh to be created is to be used in FLUENT 6.0.Import EdgeTo specify the airfoil geometry, we'll import a file containing a list of vertices along the surface and have GAMBIT join these vertices to create two edges, corresponding to the upper and lower surfaces of the airfoil. We'll then split these edges into 4 distinct edges to help us control the mesh size at the surface.Let's take a look at the vertices.dat file:The first line of the file represents the number of points on each edge (61) and the number of edges (2). The first 61 set of vertices are connected to form the edge corresponding to the upper surface; the next 61 are connected to form the edge for the lower surface.The chord length c for the geometry in vertices.dat file is 1, so x varies between 0 and 1. If you are using a different airfoil geometry specification file, note the range of x values in the file and determine the chord length c. You'll need this later on.Main Menu > File > Import > ICEM Input ...For File Name, browse and select the vertices.dat file. Select both Vertices and Edges under Geometry to Create: since these are the geometric entities we need to create. Deselect Face. Click Accept.Split EdgesNext, we will split the top and bottom edges into two edges each as shown in the figure below.We need to do this because a non-uniform grid spacing will be used for x<0.3c and a uniform grid spacing for x>0.3c. To split the top edge into HI and IG, selectMake sure Point is selected next to Split With in the Split Edge window.Select the top edge of the airfoil by Shift-clicking on it. You should see something similar to the picture below:We'll use the point at x=0.3c on the upper surface to split this edge into HI and IG. To do this, enter 0.3 for x: under Global. If your c is not equal to one, enter the value of 0.3*c instead of just 0.3.For instance, if c=4, enter 1.2. From here on, whenever you're asked to enter (some factor)*c, calculate the appropriate value for your c and enter it.You should see that the white circle has moved to the correct location on the edge.Click Apply. You will see a message saying ``Edge edge.1 was split, and edge edge.3 created'' in the Transcript window.Note the yellow marker in place of the white circle, indicating the original edge has been split into two edges with the yellow marker as its dividing point.Repeat this procedure for the lower surface to split it into HJ and JG. Use the point at x=0.3c on the lower surface to split this edge.Create Farfield BoundaryNext we'll create the farfield boundary by creating vertices and joining them appropriately to form edges.Create the following vertices by entering the coordinates under Global and the label under Label:Click the FIT TO WINDOW button to scale the display so that you can see all the vertices.As you create the edges for the farfield boundary, keep the picture of the farfield nomenclature given at the top of this step handy.Create the edge AB by selecting the vertex A followed by vertex B. Enter AB for Label. Click Apply. GAMBIT will create the edge. You will see a message saying something like "Created edge: AB'' in the Transcript window.Similarly, create the edges BC, CD, DE, EG, GA and CG. Note that you might have to zoom in on the airfoil to select vertex G correctly.Next we'll create the circular arc AF. Right-click on the Create Edge button and select Arc.In the Create Real Circular Arc men o Center will be yellow. That meansu, the box next tthat the vertex you select will be taken as the center of the arc. Select vertex G and click Apply.Now the box next to End Points will be highlighted in yellow. This means that you can now select the two vertices that form the end points of the arc. Select vertex A and then vertex F. Enter AF under Label. Click Apply.If you did this right, the arc AF will be created. If you look in the transcript window, you'll see a message saying that an edge has been created.Similarly, create an edge corresponding to arc EF.Create FacesThe edges can be joined together to form faces (which are planar surfaces in 2D). We'll create three faces: ABCGA, EDCGE and GAFEG+airfoil surface. Then we'll mesh each face.This brings up the Create Face From Wireframe menu. Recall that we had selected vertices in order to create edges. Similarly, we will select edges in order to form a face.To create the face ABCGA, select the edges AB, BC, CG, and GA and click Apply. GAMBIT will tell you that it has "Created face: face.1'' in the transcript window.Similarly, create the face EDCGE.To create the face consisting of GAFEG+airfoil surface, select the edges in the following order: AG, AF, EF, EG, and JG, HJ, HI and IG (around the airfoil in the clockwise direction). Click Apply.Step 2: Mesh Geometry in GAMBITMesh FacesWe'll mesh each of the 3 faces separately to get our final mesh. Before we mesh a face, we need to define the point distribution for each of the edges that form the face i.e. we first have to mesh the edges. We'll select the mesh stretching parameters and number of divisions for each edge based on three criteria:1.We'd like to cluster points near the airfoil since this is where the flow is modified the most; the meshresolution as we approach the farfield boundaries can become progressively coarser since the flow gradients approach zero.2.Close to the surface, we need the most resolution near the leading and trailing edges since these arecritical areas with the steepest gradients.3.We want transitions in mesh size to be smooth; large, discontinuous changes in the mesh sizesignificantly decrease the numerical accuracy.The edge mesh parameters we'll use for controlling the stretching are successive ratio, first length and last length. Each edge has a direction as indicated by the arrow in the graphics window. The successive ratio R is the ratio of the length of any two successive divisions in the arrow direction as shown below. Go to the index of the GAMBIT User Guide and look under Edge>Meshing for this figure and accompanying explanation. This help page also explains what the first and last lengths are; make sure you understand what they are.Select the edge GA. The edge will change color and an arrow and several circles will appear on the edge. This indicates that you are ready to mesh this edge. Make sure the arrow is pointing upwards. You can reverse the direction of the edge by clicking on the Reverse button in the Mesh Edges menu. Enter a ratio of 1.15. This means that each successive mesh division will be 1.15 times bigger in the direction of the arrow. Select Interval Count under Spacing. Enter 45 for Interval Count. Click Apply. GAMBIT will create 45 intervals on this edge with a successive ratio of 1.15.For edges AB and CG, we'll set the First Length (i.e. the length of the division at the start of the edge) rather than the Successive Ratio. Repeat the same steps for edges BC, AB and CG with the following specifications:Note that later we'll select the length at the trailing edge to be 0.02c so that the mesh length is continuous between IG and CG, and HG and CG.Now that the appropriate edge meshes have been specified, mesh the face ABCGA:Select the face ABCGA. The face will changecolor. You can use the defaults of Quad(i.e. quadrilaterals) and Map. Click Apply. The meshed face should look as follows:Next mesh face EDCGE in a similar fashion. The following table shows the parameters to use for the different edges:The resultant mesh should be symmetric about CG as shown in the figure below.Finally, let's mesh the face consisting of GAFEG and the airfoil surface. For edges HI and HJ on the front part of the airfoil surface, use the following parameters to create edge meshes:For edges IG and JG, we'll set the divisions to be uniform and equal to 0.02c. Use Interval Size rather than Interval Count and create the edge meshes:For edge AF, the number of divisions needs to be equal to the number of divisions on the line opposite to it i.e. the upper surface of the airfoil (this is a subtle point; chew over it). To determine the number of divisions that GAMBIT has created on edge IG, selectSelect edge IG and then Elements under Component and click Apply. This will give the total number of nodes (i.e. points) and elements (i.e. divisions) on the edge in the Transcript window. The number of divisions on edge IG is 35. (If you are using a different geometry, this number will be different; I'll refer to it as N IG). So the Interval Count for edge AF is N+N IG= 40+35= 75.HISimilarly, determine the number of divisions on edge JG. This also comes out as 35 for the current geometry. So the Interval Count for edge EF also is 75.Create the mesh for edges AF and EF with the following parameters:Mesh the face. The resultant mesh is shown below.Step 3: Specify Boundary Types in GAMBITWe'll label the boundary AFE as farfield1, ABDE as farfield2 and the airfoil surface as airfoil. Recall that these will be the names that show up under boundary zones when the mesh is read into FLUENT.Group EdgesWe'll create groups of edges and then create boundary entities from these groups.First, we will group AF and EF together.Select Edges and enter farfield1 for Label, which is the name of the group. Select the edges AF and EF.Note that GAMBIT adds the edge to the list as it is selected in the GUI.Click Apply.In the transcript window, you will see the message “Created group: farfield1 group”.Similarly, create the other two farfield groups. You should have created a total of three groups:Define Boundary TypesNow that we have grouped each of the edges into the desired groups, we can assign appropriate boundary types to these groups.Under Entity, select Groups.Select any edge belonging to the airfoil surface and that will select the airfoil group. Next to Name:, enter airfoil. Leave the Type as WALL.Click Apply.Similarly, create boundary entities corresponding to farfield1, farfield2 and farfield3 groups. Set the Type to Pressure Farfield in each case.Save Your WorkMain Menu > File > SaveExport MeshMain Menu > File > Export > Mesh...Save the file as airfoil.msh.Make sure that the Export 2d Mesh option is selected.Check to make sure that the file is createdStep 4: Set Up Problem in FLUENTLaunch FLUENTStart > Programs > Fluent Inc > FLUENT 6.0Select 2ddp from the list of options and click Run.Import FileMain Menu > File > Read > Case...Navigate to your working directory and select the airfoil.msh file. Click OK.The following should appear in the FLUENT window:Check that the displayed information is consistent with our expectations of the airfoil grid. Analyze GridGrid > Info > SizeHow many cells and nodes does the grid have?Display > GridNote what the surfaces farfield1, farfield2, etc. correspond to by selecting and plotting them in turn.Zoom into the airfoil.Where are the nodes clustered? Why?Define PropertiesDefine > Models > Solver...Under the Solver box, select Segregated.Click OK.Define > Models > ViscousSelect Inviscid under Model.Click OK.Define > Models > EnergyThe speed of sound under SSL conditions is 340 m/s so that our freestream Mach number is around 0.15. This is low enough that we'll assume that the flow is incompressible. So the energy equation can be turned off.Make sure there is no check in the box next to Energy Equation and click OK. Define > MaterialsMake sure air is selected under Fluid Materials. Set Density to constant and equal to 1.225 kg/m3.Click Change/Create.Define > Operating ConditionsWe'll work in terms of gauge pressures in this example. So set Operating Pressure to the ambient value of 101,325 Pa.Click OK.Define > Boundary ConditionsSet farfield1 and farfield2 to the velocity-inlet boundary type.For each, click Set.... Then, choose Components under Velocity Specification Method and set the x- and y-components to that for the freestream. For instance, the x-component is 50*cos(5o)=49.81.Click OK.Set farfield3 to pressure-outlet boundary type, click Set... and set the Gauge Pressure at this boundary to 0.Click OK.Step 5: Solve!Solve > Control > SolutionTake a look at the options available.Under Discretization, set Pressure to PRESTO! and Momentum to Second-Order Upwind.Click OK.Solve > Initialize > Initialize...As you may recall from the previous tutorials, this is where we set the initial guess values (the base case) for the iterative solution. Once again, we'll set these values to be the ones at the inlet. Select farfield1 under Compute From.Click Init.Solve > Monitors > Residual...Now we will set the residual values (the criteria for a good enough solution). Once again, we'll set this value to 1e-06.Solve > Monitors > Force...Under Coefficient, choose Lift. Under Options, select Print and Plot. Then, Choose airfoil under Wall Zones.Lastly, set the Force Vector components for the lift. The lift is the force perpendicular to the direction of the freestream. So to get the lift coefficient, set X to -sin(5°)=-0.0872 and Y to cos(5°)=0.9962.Click Apply for these changes to take effect.Similarly, set the Force Monitor options for the Drag force. The drag is defined as the force component in the direction of the freestream. So under Force Vector, set X to cos(5°)=0.9962 and Y to sin(5°)=0.0872. Turn on only Print for it.Report > Reference ValuesNow, set the reference values to set the base cases for our iteration. Select farfield1 under Compute From.Main Menu > File > Write > Case...Save the case file before you start the iterations.Solve > IterateWhat does the convergence plot look like?How many iterations does it take to converge?Main Menu > File > Write > Case & Data...Save case and data after you have obtained a converged solution.Step 6: Analyze ResultsPlot Pressure CoefficientPlot > XY Plot...Change the Y Axis Function to Pressure..., followed by Pressure Coefficient. Then, select airfoil under Surfaces.Click Plot.Plot Pressure ContoursDisplay > Contours...Select Pressure ... and Static Pressure from under Contours Of . Click Display .Where are the highest and lowest pressures occurring?Problem 1Consider the incompressible , inviscid airfoil calculation in FLUENT presented in class. Recall that the angle of attack, a, was 5°.Repeat the calculation for the airfoil for a = 0° and a = 10°. Save your calculation for each angle of attack as a different case file.(a) Graph the pressure coefficient (Cp ) distribution along the airfoil surface at a = 5° and a = 10° in the manner discussed in class (i.e., follow the aeronautical convention of letting Cp decrease with increasing ordinate (y -axis) values).What change do you see in the Cp distribution on the upper and lower surfaces as you increase the angle of attack?Which part of the airfoil surface contributes most to the increase in lift with increasing a? Hint: The area under the Cp vs. x curve is approximately equal to Cl .(b) Make a table of Cl and Cd values obtained for a = 0°, 5°, and 10°. Plot Cl vs.a for the three values of a. Make a linear leastsquares fit of this data and obtain the slope. Compare your result to that obtained from inviscid, thinairfoil theory:180αd 22π=dC l where a is in degrees.Problem 2Repeat the incompressible calculation at a = 5° including viscous effects . Since thewith the enhanced wall treatment option. At the farfield boundaries, set turbulence intensity=1% and turbulent length scale=0.01.(a) Graph the pressure coefficient (Cp) distribution along the airfoil surface for this calculation and the inviscid calculation done in the previous problem at a = 5°. Comment on any differences you observe.(b) Compare the Cl and Cd values obtained with the corresponding values from the inviscid calculation. Discuss briefly the similarities and differences between the two results. Copyright 2002.Cornell UniversitySibley School of Mechanical and Aerospace Engineering。
fluent计算跨音速翼型教程

c Fluent Inc. August 7, 1998
2-1
Transonic Turbulent Flow Around an Airfoil
α = 4° M∞= 0.8
1m
Figure 2.1: Problem Speci cation
Preparation
1. Copy the le
fluent_inc fluent5 tut airfoil airfoil.msh
Extra: You can use the right mouse button to check which
zone number corresponds to each boundary. If you click the right mouse button on one of the boundaries in the graphics window, its zone number, name, and type will be printed in the FLUENT console window. This feature is especially useful when you have several zones of the same type and you want to distinguish between them quickly.
3. Turn on the Spalart-Allmaras turbulence model.
De ne ,! Models ,!Viscous...
a Select the Spalart-Allmaras model and retain the default options and constants.
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
CAE联盟论坛精品讲座系列详细FLUENT实例讲座-翼型计算主讲人:流沙CAE联盟论坛总版主问题描述翼型升阻力计算是CFD最常规的应用之一。
本例计算的翼型为RAE2822,其几何参数可以查看翼型数据库。
本例计算在来流速度马赫,攻角°情况下,翼型的升阻系数及流场分布,并将计算结果与实验数据进行对比。
模型示意图如图1所示。
FLUENT前处理设置Step 1:导入计算模型以3D,双精度方式启动。
利用菜单【File】>【Read】>【Mesh…】,在弹出的文件选择对话框中选择网格文件,点击OK按钮选择文件。
如图2所示。
点击FLUENT模型树按钮General,在右侧设置面板中点击按钮Display…,在弹出的设置对话框中保持默认设置,点击Display按钮,显示网格。
如图3所示。
Step 2:检查网格采用如图4所示步骤进行网格的检查与显示。
点击FLUENT模型树节点General节点,在右侧面板中通过按钮Scale…、Check及Report Quality实现网格检查。
点击按钮Check,在命令输出按钮出现如图5所示网格统计信息。
从图中可以看出,网格尺寸分布:x轴:~50my轴:0~z轴:-50~50m符合尺寸要求,无需进行尺寸缩放。
最小网格体积参数minimum volume为,为大于0的值,符合计算要求。
Step 3:General设置点击模型树节点General,在右侧设置面板中Solver下设置求解器为Density-Based,如图6所示。
小提示:对于高速可压缩流场计算,常常使用密度基求解器。
Step 4:Models设置使用SST k-w湍流模型,并且激活能量方程。
1、激活SST k-w湍流模型如图6-7所示,点击模型树节点Models,在右侧面板中的models列表项中鼠标双击Viscous-laminar,弹出如图6-8所示粘性模型设置对话框,在model选项中选择k-omega(2 eqn),并在k-omega Model选项中选择选项SST,其它参数保持默认。
小技巧:对于外流场模型,若壁面附近流场非常重要,则SST k-w模型是理想的选择。
该湍流模型可以求解粘性子层,不过对网格要求较高,壁面附近需要非常细密的网格。
2、激活能量方程在图7所示面板中鼠标双击列表项Eneergy-Off,弹出能量方程设置面板,在面板中激活Energy Equation选项。
Step 5:Materials设置设置气体密度为理想气体类型。
如图9所示,点击FLUENT模型树节点Materials,在右侧设置面板中选择材料air,点击按钮Create/Edit…,弹出材料设置对话框。
如图10所示。
设置密度Density选项为ideal-gas,设置粘性Viscosity选项为sutherland,在弹出的相应面板中采取默认设置。
点击Change/Create按钮完成材料属性的编辑。
小提示:ideal-gas采用的是理想气体状态方程,能够反应压力与密度的关系,可以模拟流体的可压缩性。
对于高速可压缩流动问题,通常其流体物性与温度关系较大,本例进行了简化,设置其比热及热传导率为定值。
Step 6:Cell Zone Conditions设置在Cell Zone Conditions中设置参考压力为0。
如图11所示,点击模型树节点Cell Zone Conditions,在右侧设置面板中点击按钮Operating Conditions…,弹出如图12所示的设置对话框。
在对话框中设置参数Operating Pressure为0。
小技巧:设置操作压力为零意味着在边界条件中设置的压力均为绝对压力。
Step 7:Boundary Conditions设置设置入口边界inlet的边界类型为Pressure Far-Field。
设置壁面边界airfoil的边界类型为Wall。
设置对称边界symmetry的边界类型为Symmetry。
1、设置入口边界inlet如图13所示,点击模型树节点Boundary Conditions,在右侧面板中Zone选项中选择列表项inlet,设置边界类型Type为pressure-far-field,点击Edit…按钮在弹出的参数设置对话框中设置入口边界参数。
如图14所示。
在Momentum标签页中,设置表压Gauge Pressure为11111Pa,设置马赫数Mach Number为,设置速度向量为直角坐标方式Cartesian。
设置方向向量为(,0,)。
该向量为通过攻角°计算获得。
°=,°=。
设置湍流指定方式Specification Method为Intensity and Viscosity Ratio,指定湍流强度Turbulent Intensity为1%,湍流粘度比Turbulent Viscosity Ratio为1。
切换至Thermal标签页,设置温度Temperature为。
如图15所示。
2、设置airfoil边界及symmetry边界设置airfoil边界类型为Wall,保持参数默认,即使用无滑移光滑绝热壁面。
修改symmetry边界类型为Symmetry。
Step 8:Reference Value设置参考值主要用于升阻系数的计算。
如图16所示设置。
点击模型树节点Reference Values,在右侧面板中Computer from选择inlet,软件会自动对下方的参数进行填充。
用户需要确保Area参数值为。
软件利用参数值进行升力系数及阻力系数的计算:式中,CD为阻力系数,CL为升力系数。
Fstream为水平分力,Flateral为垂直分力。
Step 9:Solution Methods设置如图17所示,点击模型树节点Solution Methods,在右侧面板中设置求解方法。
如图所示,使用Implicit及Roe-FDS求解方法,修改Turbulent Kinetic Energy与Specific Dissipation Rat为Second Order Upwind,其它参数保持默认设置。
Step 10:Solution Controls设置求解控制参数采用默认设置。
该面板中的设置主要用于控制收敛性,通常软件会根据用户设置的模型及边界条件对控制参数进行一定的优化,用户往往无需进行设定。
在该面板中主要设置物理量的亚松弛因子。
增大亚松弛因子能提高收敛速度,但是会降低稳定性。
Step 11:Monitor设置可以定义升力及阻力系数监视器,以观察这些物理量随迭代进行的变化情况。
1、定义阻力监视器如图18所示,鼠标点击模型树节点Monitors,在右侧设置面板中点击如图所示Create按钮下的Drag…菜单,弹出如图19所示的设置对话框。
按如图19所示,定义阻力监视器。
2、升力监视器升力监视器定义步骤与阻力监视器相同,所不同的是力向量Force Vector改为[,0,。
Step 12:Solution Initialization设置以入口inlet边界条件进行初始化。
如图20及图21所示。
Step 13:Run Calculation设置点击FLUENT模型树节点Run Calculation,右侧面板设置如图21所示。
设置Number of Iterations为0,激活选项Solution Steering,在选项Flow Type为Transonic,激活选项Use FMG Initialization,点击按钮Calculate进行FMG初始化。
小技巧:对于航空外流问题,采用FMG初始化有助于提高收敛性。
设置Number of Iterations为900,取消激活选项Use FMG Initialization,点击Calculation按钮进行迭代计算。
结果后处理Step 1:升阻系数监控曲线图22与图23分别为升力系数与阻力系数监控曲线。
从图中可以看出,随着迭代次数的增加,升力系数及阻力系数逐渐趋于稳定。
可以认为计算达到收敛。
图24为迭代输出结果部分截图,从图中可以看出,升力系数约为,阻力系数约为。
Step 2:沿壁面的压力系数分布点击模型树节点Plot,在右侧面板中选择列表项XY Plot,弹出面板如图25所示。
激活选项Node Values与Position on X Axis,设置Plot Direction为(1,0,0),设置Y Axis Function为Pressure与Pressure Coefficient,设置X Axis Function为Direction Vector,选择Surface为airfoil。
点击按钮Load File…,在弹出的文件选择对话框中选择试验数据文件。
点击Plot按钮显示曲线。
压力系数分布如图28所示。
点击Axes按钮,弹出如图26所示坐标轴样式设置对话框。
激活选择Y Axis,设置Number Format的Type为float,设置精度Precision为2。
点击Curve按钮设置曲线样式。
如图27所示。
对话框中Curve#中选择曲线编号,Line Style 设置曲线的样式:包括曲线的线型(Pattern)、颜色(Color)及线宽(weight)。
在Maker Style中设置曲线上标记点的样式,包括参数symbol、Color及size。
设置本次计算的曲线为实线,蓝色,线宽为2。
如图所示。
图28所示为压力系数分布曲线及实验数据分布。
从图中可以看出,数值仿真计算结果与实验数据吻合较好。
用户可以加密网格以提高计算精度。
Step 3:修改视图方向本例中视图方向如图29上图所示。
用户往往习惯以来流方向从左至右显示,因此需要修改视图方向以调整模型观察方向,如图29下图所示。
利用菜单【Display】>【View…】弹出如图30所示视图定义对话框。
点击Views对话框中的按钮Camera…,在弹出的对话框中设置Camera为Up Vector,设置向量为(0,0,-1),点击Apply及Close按钮确认并关闭对话框。
返回至views对话框中点击Save按钮保存视图,点击Apply按钮确认视图选择。
调整视图后的图形显示框中模型显示如图29右图所示。
Step 4:查看马赫数分布点击模型树节点Graphics and Animations,在右侧面板Graphics中选择列表项Contours,点击Set Up按钮进入云图设置对话框。
如图31所示,在Options中确保已选中选项Filled、Node Value、Global Range及Auto Range,同时设置Contours of组合框内容为Velocity…及Mach Number,选择Surfaces为Symmetry。
