数字电路与系统设计(第二版) 第1章

合集下载

数字电路与逻辑设计教程-第1章

上一页下一页返回
1.2 数制和码制
【例1-4】求十进制数(26)10所对应的二进制数。
因此(26)10=(11010)2。
上一页下一页返回
1.2 数制和码制
【例1-5】求十进制数(357 ) 10所对应的八进制数。解
因此(357 )10=(545)8。
上一页下一页返回
1.2 数制和码制
上一节介绍了数字信号的两种取值，实际生活中的数字表示大多采用进位计数制。
下一页返回
1.2 数制和码制
1.2.1 进位计数制与常用计数制
用数字量表示物理量大小时，仅用一位数码往往不够用，经常需要用进位计数的方法组成多位数码表示。把多位数码中每一位的构成方法以及从低位到高位的进位规则称为计数制。在生产实践中除了人们最熟悉的十进制以外，还大量使用各种不同的进位计数制，如八进制、十六进制等。在数字设备中，机器只认识二进制代码，由于二进制代码书写长，所以在数字设备中又常采用八进制代码或十六进制代码。
上一页下一页返回
1.2 数制和码制
任何进制数的值都可以表示为该进制数中各位数字符号值与相应权乘积的累加和形式，该形式称为按权展开的多项式之和。一个J进制数(N为按权展开的多项式的普遍形式可表示为 :
式中，K为任意进制数中第i位的系数，可以为0~ (J-1)数码中的任何一个;i是数字符号所处位置的序号;m和n为整数，m为小数部分位数(取负整数)，n为整数部分位数(取正整数);.J为进位基数，Ji为第i位的权值。例如，十进制数(123.75 )10表示为:
第1章微型计算机系统概述
1.1 数字电路概述 1.2 数制和码制 1.3 逻辑代数基础本章小结
1.1 数字电路概述

数字电路与逻辑设计(第二版)章图文 (2)

第2章组合逻辑电路
第2章组合逻辑电路
2.1 集成门电路 2.2 组合逻辑电路的分析和设计 2.3 组合逻辑电路中的竞争-冒险
第2章组合逻辑电路
2.1 集成门电路
2.1.1 TTL门电路 TTL门电路由双极型三极管构成,它的特点是速度
快、抗静电能力强、集成度低、功耗大,目前广泛应用于中、小规模集成电路中。TTL门电路有74（商用）和54（军用）两大系列,每个系列中又有若干子系列,例如，74系列包含如下基本子系列：
4)传输延时tP 传输延时tP指输入变化引起输出变化所需的时间,它是衡量逻辑电路工作速度的重要指标。传输延时越短, 工作速度越快,工作频率越高。tPHL指输出由高电平变为低电平时,输入脉冲的指定参考点（一般为中点）到输出脉冲的相应指定参考点的时间。tPLH指输出由低电平变为高电平时,输入脉冲的指定参考点到输出脉冲的相应指定参考点的时间。标准TTL系列门电路典型的传输延时为11ns;高速TTL系列门电路典型的传输延时为3.3ns。HCT系列CMOS门电路的传输延时为7ns;AC 系列CMOS门电路的传输延时为5ns;ALVC系列CMOS 门电路的传输延时为3ns。
第2章组合逻辑电路
图2―2和图2―3分别给出了TTL电路和CMOS电路的输入/输出逻辑电平。
当输入电平在UIL(max)和UIH(min)之间时,逻辑电路可能把它当作0,也可能把它当作1，而当逻辑电路因所接负载过多等原因不能正常工作时,高电平输出可能低于 UOH(min),低电平输出可能高于UOL(max)。
第2章组合逻辑电路
74AC和74ACT:先进CMOS(Advanced CMOS)。 74AHC和74AHCT:先进高速CMOS(Advanced High speed

(整理)集成电路原理学习指南-第二版

3.17
沟道等效电阻
（1）与W/L反比，
（2）与电压有关，
（3）VDD大的时候较小（饱和工作区）
（4）VDD接近Vt的时候急剧增大
（5）一般使用工作区平均电阻
掌握
3.18
电阻的近似
平均电阻，并估算其误差（保守估计还是过估计）
掌握
3.19
结构电容
栅电容，覆盖电容
掌握
3.20
沟道电容
在不同工作区域的变化和原因，在阈值附近最小
f=Cext/Cint=Cext/γCg，尺寸决定电容，所以也是扇出尺寸，为工艺决定的系数，代表自电容与栅电容的关系
掌握
5.13
反相器链的最优尺寸设计
每一级为前后级的几何平均
扇出系数公式（5.35），公式（5.36）
掌握
5.14
最佳等效扇出
图5.21（pp 152），一般取4
掌握
5.15
上升下降时间对延时的影响
了解
3.26
电容估算
（1）栅电容，扩散电容大致相当（定义单位NMOS和PMOS的栅电容为C）
（2）它们随沟道宽度等比增加（kC）
（3）最小晶体管C值可初略估计为1fF/um宽度（65nm工艺，宽0.1um晶体管的C值约为0.1fF）
[Weste，4.3.2]
掌握
第四章导线
序号
概念
知识点和关键词
掌握程度
掌握
3.13
MOS IV特性
画出IV图，标出工作区，图3.24（pp 74）
掌握并会定性画图
3.14
手工分析的局限
在电阻区和过度区之间的区域偏差较大
了解
3.15
设计测试点验证IV
知道晶体管几个端口的电压，固定哪个，量哪个电流，可以提取以上列出的某个参数。

数字电路逻辑设计(第二版) 王毓银电子科技大学

3.5.3 CMOS传输门
3.5.4 CMOS逻辑门电路
3.5.5 BiCMOS门电路
3.5.6 CMOS电路的正确使用方法
3.6 VHDL描述逻辑门电路
3.6.1 VHDL描述电路的基本方法
3.6.2 VHDL描述逻辑门电路
习题
第4章组合逻辑电路
4.1 组合逻辑电路分析
6.4.1 设计给定序列信号的产生电路
6.4.2 根据序列循环长度M的要求设计发生器电路
6.5 时序逻辑电路的VHDL描述
6.5.1 移位寄存器的VHDL描述
6.5.2 计数器的VHDL描述
习题
第7章半导体存储器
7.1 概述
7.1.1 半导体存储器的特点与应用
5.3 主从触发器
5.3.1 主从触发器基本原理
5.3.2 主从J-K触发器主触发器的一次翻转现象
5.3.3 主从J-K触发器集成单元
5.3.4 集成主从J-K触发器的脉冲工作特性
5.4 边沿触发器
5.4.1 维持一阻塞触发器
5.4.2 下降沿触发的边沿触发器
10.2.6 DAC的转换精度与转换速度
10.3 模数转换器（ADC）
10.3.1 模数转换基本原理
10.3.2 并联比较型ADC
10.3.3 逐次逼近型ADC
10.3.4 双积分型ADC
10.4 集成ADC及其应用举例
双积分型集成ADC
10.4.2 逐次逼近型集成ADC
2.1.3 真值表与逻辑函数
2.1.4 逻辑函数相等
2.1.5 三个规则
2.1.6 常用公式
2.1.7 逻辑函数的标准形式

数字电路与逻辑设计微课版(第一章数字电路与逻辑设计基础)教案

第一章数字电路与逻辑设计基础本章的主要知识点包括数制及其转换、二进制的算术运算、BCD码和可靠性编码等。

1.参考学时2学时（总学时32课时，课时为48课时可分配4学时）。

2.教学目标（能力要求）●系统梳理半导体与微电子技术发展的历史，激发学生专业热情，结合我国计算机发展面临的卡脖子现状，鼓励学生积极投身信息成业自主可控；●学生可解释数字系统的概念、类型及研究方法；●学生能阐述数制的基本特点，可在不同数制之间进行数字的转换；●学生能理解带符号二进制数的代码表示，能将真值和原码、反码、补码的进行转换；●学生能熟记几种常用的编码（8421码、2421码、5421码、余三码），说明有权码和无权码的区别，能阐述不同编码的特点和特性；●学生能阐述奇偶校验码和格雷码的工作原理与主要特征，并能利用相关原理进行二进制和格雷码的转换，能根据信息码生成校验码，并能根据信息码和校验码辨别数据是否可靠。

3.教学重点●BCD码●奇偶校验码●格雷码4.教学难点●理解不同BCD码的编码方案及相关特征●理解可靠性编码方案、验证的原理以及使用方法。

5.教学主要内容（1）课程概述（15分钟）➢科技革命促生互联网时代➢半导体与微电子技术发展历程➢课程性质、内容与学习方法（2）芯片与数字电路（20分钟）➢数字信号和模拟信号➢数字逻辑电路的特点➢数字逻辑电路的分类➢数字逻辑电路的研究方法（3）数制及其转换（5分钟）➢进位计数值的概念和基本要素➢二进制和十进制的相互转换➢二进制和八进制数的相互转换➢二进制和十六进制数的相互转换（4）二进制数的算术运算（5分钟）➢无符号二进制数的算术运算➢带符号二进制数的机器码表示➢带符号二进制数的算术运算（5）BCD码（20分钟）➢有权码和无权码的区别➢8421码的编码规律及和十进制数的转换➢2421码的编码规律及和十进制数的转换➢5421码的编码规律及和十进制数的转换➢余三码的编码规律及和十进制数的转换（6）奇偶校验码（15分钟）➢奇校验和偶校验的概念➢奇校验和偶校验校验位的生成方法和校验方法➢奇校验和偶校验的特点（7）格雷码（10分钟）➢格雷码的特点和用途➢格雷码和二进制数的相互转换6.教学过程与方法（1）课程概述（15分钟）➢科技革命促生互联网时代以习总书记的讲话作为整个课程的导入，说明科技发展是强国必有之路，穿插不同国家崛起的历史，结合第一次工业革命、第二次工业革命，推出目前进入的互联网时代，结合中美贸易战事件，引导学生积极投身国产IT生态的建设。

数字集成电路--电路、系统与设计（第二版）课后练习题第六.

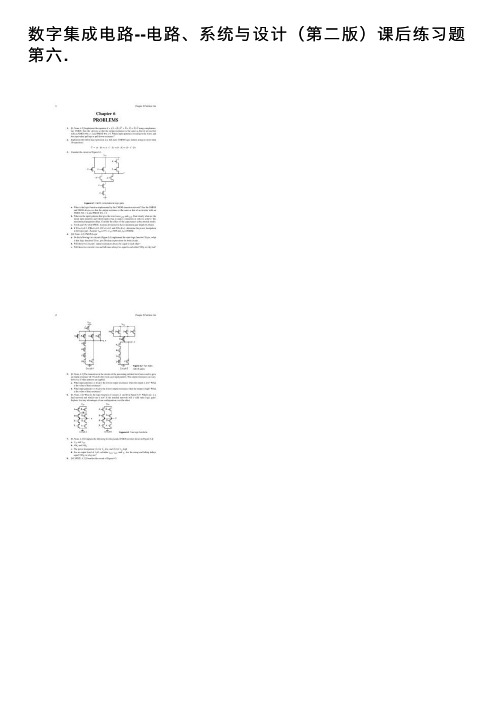
数字集成电路--电路、系统与设计（第⼆版）课后练习题第六.Digital Integrated Circuits - 2nd Ed 11 DESIGN PROJECT Design, lay out, and simulate a CMOS four-input XOR gate in the standard 0.25 micron CMOS process. You can choose any logic circuit style, and you are free to choose how many stages of logic to use: you could use one large logic gate or a combination of smaller logic gates. The supply voltage is set at 2.5 V! Your circuit must drive an external 20 fF load in addition to whatever internal parasitics are present in your circuit. The primary design objective is to minimize the propagation delay of the worst-case transition for your circuit. The secondary objective is to minimize the area of the layout. At the very worst, your design must have a propagation delay of no more than 0.5 ns and occupy an area of no more than 500 square microns, but the faster and smaller your circuit, the better. Be aware that, when using dynamic logic, the precharge time should be made part of the delay. The design will be graded on themagnitude of A × tp2, the product of the area of your design and the square of the delay for the worst-case transition.。

数字集成电路-电路系统与设计第二版课程设计

数字集成电路-电路系统与设计第二版课程设计
一、课程设计介绍
数字集成电路是现代电路设计中的重要组成部分，也是计算机科学与工程的重要分支。

本课程设计旨在通过对数字集成电路的系统与设计进行探究，并结合具体的案例来设计和实现数字集成电路，使学生能够熟悉数字集成电路的基本原理、设计方法和实现技术。

本课程设计主要包含以下内容：
1.数值系统和编码
2.逻辑功能设计：组合逻辑电路和时序逻辑电路
3.集成电路设计方法和流程
4.VHDL和FPGA实现数字逻辑电路
5.数字信号处理器
通过本次课程设计，学生将掌握数字集成电路的系统性设计思路和实现方法，具备数字电路设计的基本能力和实际操作技术，能够针对具体应用场景提出解决方案，实现数字电路的设计、验证和调试。

二、课程设计要求
1. 课程设计题目
本次课程设计的题目为“4位计数器设计”。

2. 软件工具
VHDL编程软件和EDA工具
1。

【精品】数字集成电路电路、系统与设计第二版课后练习题第六章CMOS组合逻辑门的设计

【精品】数字集成电路--电路、系统与设计(第二版)课后练习题第六章CMOS组合逻辑门的设计第六章 CMOS组合逻辑门的设计1.为什么CMOS电路逻辑门的输入端和输出端都要连接到电源电压？CMOS电路采用了MOSFET（金属氧化物半导体场效应管）作为开关元件，其中N沟道MOSFET（NMOS）和P沟道MOSFET（PMOS）分别用于实现逻辑门的输入和输出。

NMOS和PMOS都需要连接到电源电压，以使其能够正常工作。

输入端连接到电源电压可以确保信号在逻辑门中正常传递，输出端连接到电源电压可以确保输出信号的正确性和稳定性。

2.为什么在CMOS逻辑门中要使用两个互补的MOSFET？CMOS逻辑门中使用两个互补的MOSFET是为了实现高度抗干扰的逻辑功能。

其中，NMOS和PMOS分别用于实现逻辑门的输入和输出。

NMOS和PMOS的工作原理互补，即当NMOS导通时，PMOS截止，当PMOS导通时，NMOS截止。

这样的设计可以在逻辑门的输出上提供高电平和低电平的稳定性，从而提高逻辑门的抗干扰能力。

3.CMOS逻辑门的输入电压范围是多少？CMOS逻辑门的输入电压范围通常是在0V至电源电压之间，即在低电平和高电平之间。

在CMOS逻辑门中，低电平通常定义为输入电压小于0.3Vdd（电源电压的30%），而高电平通常定义为输入电压大于0.7Vdd（电源电压的70%）。

4.如何设计一个基本的CMOS逻辑门？一个基本的CMOS逻辑门可以由一个NMOS和一个PMOS组成。

其中，NMOS的源极连接到地，栅极连接到逻辑门的输入，漏极连接到PMOS的漏极；PMOS的源极连接到电源电压，栅极连接到逻辑门的输入，漏极连接到输出。

这样的设计可以实现逻辑门的基本功能。

5.如何提高CMOS逻辑门的速度？可以采取以下方法来提高CMOS逻辑门的速度：•减小晶体管的尺寸：缩小晶体管的尺寸可以减小晶体管的电容和电阻，从而提高逻辑门的响应速度。

•优化电源电压：增加电源电压可以提高晶体管的驱动能力，从而加快逻辑门的开关速度。

《verilog_数字系统设计课程》(第二版)思考题答案

绪论1.什么是信号处理电路？它通常由哪两大部分组成？信号处理电路是进行一些复杂的数字运算和数据处理，并且又有实时响应要求的电路。

它通常有高速数据通道接口和高速算法电路两大部分组成。

2.为什么要设计专用的信号处理电路？因为有的数字信号处理对时间的要求非常苛刻，以至于用高速的通用处理器也无法在规定的时间内完成必要的运算。

通用微处理器芯片是为一般目的而设计的，运算的步骤必须通过程序编译后生成的机器码指令加载到存储器中，然后在微处理器芯片控制下，按时钟的节拍，逐条取出指令分析指令和执行指令，直到程序的结束。

微处理器芯片中的内部总线和运算部件也是为通用目的而设计，即使是专为信号处理而设计的通用微处理器，因为它的通用性也不可能为某一特殊的算法来设计一系列的专用的运算电路而且其内部总线的宽度也不能随便的改变，只有通过改变程序，才能实现这个特殊的算法，因而其算法速度也受到限制所以要设计专用的信号处理电路。

3.什么是实时处理系统？实时处理系统是具有实时响应的处理系统。

4.为什么要用硬件描述语言来设计复杂的算法逻辑电路？因为现代复杂数字逻辑系统的设计都是借助于EDA工具完成的，无论电路系统的仿真和综合都需要掌握硬件描述语言。

5.能不能完全用C语言来代替硬件描述语言进行算法逻辑电路的设计？不能，因为基础算法的描述和验证通常用C语言来做。

如果要设计一个专用的电路来进行这种对速度有要求的实时数据处理，除了以上C语言外，还须编写硬件描述语言程序进行仿真以便从电路结构上保证算法能在规定的时间内完成，并能通过与前端和后端的设备接口正确无误地交换数据。

6.为什么在算法逻辑电路的设计中需要用C语言和硬件描述语言配合使用来提高设计效率？首先C语言很灵活，查错功能强，还可以通过PLI编写自己的系统任务，并直接与硬件仿真器结合使用。

C语言是目前世界上应用最为广泛的一种编程语言，因而C程序的设计环境比Verilog HDL更完整，此外，C语言有可靠地编译环境，语法完备，缺陷缺少，应用于许多的领域。

数字集成电路--电路、系统与设计(第二版)课后练习题第五章 CMOS反相器

C H A P T E R5T H E C M O S I N V E R T E R Quantification of integrity,performance,and energy metrics of an inverterOptimization of an inverter design5.1Exercises and Design Problems5.2The Static CMOS Inverter—An IntuitivePerspective5.3Evaluating the Robustness of the CMOSInverter:The Static Behavior5.3.1Switching Threshold5.3.2Noise Margins5.3.3Robustness Revisited5.4Performance of CMOS Inverter:The DynamicBehavior5.4.1Computing the Capacitances5.4.2Propagation Delay:First-OrderAnalysis5.4.3Propagation Delay from a DesignPerspective5.5Power,Energy,and Energy-Delay5.5.1Dynamic Power Consumption5.5.2Static Consumption5.5.3Putting It All Together5.5.4Analyzing Power Consumption UsingSPICE5.6Perspective:Technology Scaling and itsImpact on the Inverter Metrics180Section 5.1Exercises and Design Problems 1815.1Exercises and Design Problems1.[M,SPICE,3.3.2]The layout of a static CMOS inverter is given in Figure 5.1.(λ=0.125µm).a.Determine the sizes of the NMOS and PMOS transistors.b.Plot the VTC (using HSPICE)and derive its parameters (V OH ,V OL ,V M ,V IH ,and V IL ).c.Is the VTC affected when the output of the gates is connected to the inputs of 4similargates?.d.Resize the inverter to achieve a switching threshold of approximately 0.75V .Do not lay-out the new inverter,use HSPICE for your simulations.How are the noise margins affected by this modification?2.Figure 5.2shows a piecewise linear approximation for the VTC.The transition region isapproximated by a straight line with a slope equal to the inverter gain at V M .The intersectionof this line with the V OH and the V OL lines defines V IH and V IL .a.The noise margins of a CMOS inverter are highly dependent on the sizing ratio,r =k p /k n ,of the NMOS and PMOS e HSPICE with V Tn =|V Tp |to determine the valueof r that results in equal noise margins?Give a qualitative explanation.b.Section 5.3.2of the text uses this piecewise linear approximation to derive simplifiedexpressions for NM H and NM L in terms of the inverter gain.The derivation of the gain isbased on the assumption that both the NMOS and the PMOS devices are velocity saturatedat V M .For what range of r is this assumption valid?What is the resulting range of V M ?c.Derive expressions for the inverter gain at V M for the cases when the sizing ratio is justabove and just below the limits of the range where both devices are velocity saturated.What are the operating regions of the NMOS and the PMOS for each case?Consider theeffect of channel-length modulation by using the following expression for the small-signalresistance in the saturation region:r o,sat =1/(λI D ).Figure 5.1CMOS inverter layout.InOutGND V DD =2.5V.Poly Metal1NMOSPMOSPolyMetal12λ182THE CMOS INVERTER Chapter 53.[M,SPICE,3.3.2]Figure 5.3shows an NMOS inverter with resistive load.a.Qualitatively discuss why this circuit behaves as an inverter.b.Find V OH and V OL calculate V IH and V IL .c.Find NM L and NM H ,and plot the VTC using HSPICE.d.Compute the average power dissipation for:(i)V in =0V and (ii)V in =2.5Ve HSPICE to sketch the VTCs for R L =37k,75k,and 150k on a single graph.ment on the relationship between the critical VTC voltages (i.e.,V OL ,V OH ,V IL ,V IH )and the load resistance,R L .g.Do high or low impedance loads seem to produce more ideal inverter characteristics?4.[E,None,3.3.3]For the inverter of Figure 5.3and an output load of 3pF:a.Calculate t plh ,t phl ,and t p .b.Are the rising and falling delays equal?Why or why not?pute the static and dynamic power dissipation assuming the gate is clocked as fast as possible.5.The next figure shows two implementations of MOS inverters.The first inverter uses onlyNMOS transistors.V OH V OL inV outFigure 5.2A different approach to derive V IL and V IH .V outV in M 1W/L =1.5/0.5+2.5VFigure 5.3Resistive-load inverterR L =75k ΩSection 5.1Exercises and Design Problems183a.Calculate V OH ,V OL ,V M for each case.e HSPICE to obtain the two VTCs.You must assume certain values for the source/drain areas and perimeters since there is no layout.For our scalable CMOS process,λ =0.125μm,and the source/drain extensions are 5λfor the PMOS;for the NMOS the source/drain contact regions are 5λx5λ.c.Find V IH ,V IL ,NM L and NM H for each inverter and comment on the results.How can you increase the noise margins and reduce the undefined region?ment on the differences in the VTCs,robustness and regeneration of each inverter.6.Consider the following NMOS inverter.Assume that the bulk terminals of all NMOS deviceare connected to GND.Assume that the input IN has a 0V to 2.5V swing.a.Set up the equation(s)to compute the voltage on node x .Assume γ=0.5.b.What are the modes of operation of device M2?Assume γ=0.c.What is the value on the output node OUT for the case when IN =0V?Assume γ=0.d.Assuming γ=0,derive an expression for the switching threshold (V M )of the inverter.Recall that the switching threshold is the point where V IN =V OUT .Assume that the devicesizes for M1,M2and M3are (W/L)1,(W/L)2,and (W/L)3respectively.What are the limitson the switching threshold?For this,consider two cases:i)(W/L)1>>(W/L)2V DD =2.5V V IN V OUTV DD =2.5V V IN V OUT M 2M 1M 4M 3W/L=0.375/0.25W/L=0.75/0.25W/L=0.375/0.25W/L=0.75/0.25Figure 5.4Inverter ImplementationsV DD =2.5V OUTM1IN M2M3V DD =2.5Vx184THE CMOS INVERTER Chapter 5ii)(W/L)2>>(W/L)17.Consider the circuit in Figure 5.5.Device M1is a standard NMOS device.Device M2has allthe same properties as M1,except that its device threshold voltage is negative and has a valueof -0.4V.Assume that all the current equations and inequality equations (to determine themode of operation)for the depletion device M2are the same as a regular NMOS.Assume thatthe input IN has a 0V to 2.5V swing.a.Device M2has its gate terminal connected to its source terminal.If V IN =0V ,what is the output voltage?In steady state,what is the mode of operation of device M2for this input?pute the output voltage for V IN =2.5V .You may assume that V OUT is small to simplify your calculation.In steady state,what is the mode of operation of device M2for this input?c.Assuming Pr (IN =0)=0.3,what is the static power dissipation of this circuit?8.[M,None,3.3.3]An NMOS transistor is used to charge a large capacitor,as shown in Figure5.6.a.Determine the t pLH of this circuit,assuming an ideal step from 0to 2.5V at the input node.b.Assume that a resistor R S of 5k Ωis used to discharge the capacitance to ground.Deter-mine t pHL .c.Determine how much energy is taken from the supply during the charging of the capacitor.How much of this is dissipated in M1.How much is dissipated in the pull-down resistanceduring discharge?How does this change when R S is reduced to 1k Ω.d.The NMOS transistor is replaced by a PMOS device,sized so that k p is equal to the k n ofthe original NMOS.Will the resulting structure be faster?Explain why or why not.9.The circuit in Figure 5.7is known as the source follower configuration.It achieves a DC levelshift between the input and the output.The value of this shift is determined by the current I 0.Assume x d =0,γ=0.4,2|φf |=0.6V ,V T 0=0.43V ,k n ’=115μA/V 2and λ=0.V DD =2.5VOUTM1(4μm/1μm)IN M2(2μm/1μm),V Tn =-0.4VFigure 5.5A depletion load NMOSinverterV DD =2.5VOutFigure 5.6Circuit diagram with annotated W/L ratios=5pFSection 5.1Exercises and Design Problems 185a.Suppose we want the nominal level shift between V i and V o to be 0.6V in the circuit in Figure 5.7(a).Neglecting the backgate effect,calculate the width of M2to provide this level shift (Hint:first relate V i to V o in terms of I o ).b.Now assume that an ideal current source replaces M2(Figure 5.7(b)).The NMOS transis-tor M1experiences a shift in V T due to the backgate effect.Find V T as a function of V o for V o ranging from 0to 2.5V with 0.5V intervals.Plot V T vs.V oc.Plot V o vs.V i as V o varies from 0to 2.5V with 0.5V intervals.Plot two curves:one neglecting the body effect and one accounting for it.How does the body effect influence the operation of the level converter?d.At V o (with body effect)=2.5V,find V o (ideal)and thus determine the maximum error introduced by the body effect.10.For this problem assume:V DD =2.5V ,W P /L =1.25/0.25,W N /L =0.375/0.25,L =L eff =0.25μm (i.e.x d =0μm),C L =C inv-gate ,k n ’=115μA/V 2,k p ’=-30μA/V 2,V tn0=|V tp0|=0.4V,λ =0V -1, γ=0.4,2|φf |=0.6V ,and t ox =e the HSPICE model parameters for parasitic capacitance given below (i.e.C gd0,C j ,C jsw ),and assume that V SB =0V for all problems except part (e).Figure 5.7NMOS source follower configuration V DD =2.5V V iV oV DD =2.5VV i V oV bias =(a)(b)I o1um/0.25um M1186THE CMOS INVERTER Chapter 5##Parasitic Capacitance Parameters (F/m)##NMOS:CGDO=3.11x10-10,CGSO=3.11x10-10,CJ=2.02x10-3,CJSW=2.75x10-10PMOS:CGDO=2.68x10-10,CGSO=2.68x10-10,CJ=1.93x10-3,CJSW=2.23x10-10a.What is the V m for this inverter?b.What is the effective load capacitance C Leff of this inverter?(include parasitic capacitance,refer to the text for K eq and m .)Hint:You must assume certain values for the source/drain areas and perimeters since there is no layout.For our scalable CMOS process,λ =0.125μm,and the source/drain extensions are 5λfor the PMOS;for the NMOS the source/drain contact regions are 5λx5λ.c.Calculate t PHL ,t PLH assuming the result of (b)is ‘C Leff =6.5fF’.(Assume an ideal step input,i.e.t rise =t fall =0.Do this part by computing the average current used to charge/dis-charge C Leff .)d.Find (W p /W n )such that t PHL =t PLH .e.Suppose we increase the width of the transistors to reduce the t PHL ,t PLH .Do we get a pro-portional decrease in the delay times?Justify your answer.f.Suppose V SB =1V,what is the value of V tn ,V tp ,V m ?How does this qualitatively affect C Leff ?ing Hspice answer the following questions.a.Simulate the circuit in Problem 10and measure t P and the average power for input V in :pulse(0V DD 5n 0.1n 0.1n 9n 20n),as V DD varies from 1V -2.5V with a 0.25V interval.[t P =(t PHL +t PLH )/2].Using this data,plot ‘t P vs.V DD ’,and ‘Power vs.V DD ’.Specify AS,AD,PS,PD in your spice deck,and manually add C L =6.5fF.Set V SB =0Vfor this problem.b.For Vdd equal to 2.5V determine the maximum fan-out of identical inverters this gate candrive before its delay becomes larger than 2ns.c.Simulate the same circuit for a set of ‘pulse’inputs with rise and fall times of t in_rise,fall =1ns,2ns,5ns,10ns,20ns.For each input,measure (1)the rise and fall times t out_rise andV DD =2.5VV IN V OUTC L =C inv-gateL =L P =L N =0.25μmV SB-+(W p /W n =1.25/0.375)Figure 5.8CMOS inverter with capacitiveSection 5.1Exercises and Design Problems 187t out_fall of the inverter output,(2)the total energy lost E total ,and (3)the energy lost due to short circuit current E short .Using this data,prepare a plot of (1)(t out_rise +t out_fall )/2vs.t in_rise,fall ,(2)E total vs.t in_rise,fall ,(3)E short vs.t in_rise,fall and (4)E short /E total vs.t in_rise,fall.d.Provide simple explanations for:(i)Why the slope for (1)is less than 1?(ii)Why E short increases with t in_rise,fall ?(iii)Why E total increases with t in_rise,fall ?12.Consider the low swing driver of Figure 5.9:a.What is the voltage swing on the output node (V out )?Assume γ=0.b.Estimate (i)the energy drawn from the supply and (ii)energy dissipated for a 0V to 2.5V transition at the input.Assume that the rise and fall times at the input are 0.Repeat the analysis for a 2.5V to 0V transition at the input.pute t pLH (i.e.the time to transition from V OL to (V OH +V OL )/2).Assume the input rise time to be 0.V OL is the output voltage with the input at 0V and V OH is the output volt-age with the input at 2.5V .pute V OH taking into account body effect.Assume γ =0.5V 1/2for both NMOS and PMOS.13.Consider the following low swing driver consisting of NMOS devices M1and M2.Assumean NWELL implementation.Assume that the inputs IN and IN have a 0V to 2.5V swing andthat V IN =0V when V IN =2.5V and vice-versa.Also assume that there is no skew between INand IN (i.e.,the inverter delay to derive IN from IN is zero).a.What voltage is the bulk terminal of M2connected to?V in V out V DD =2.5V W L 3μm 0.25μm =p 2.5V0V C L =100fFW L 1.5μm 0.25μm=n Figure 5.9Low Swing DriverV LOW =0.5VOutM1ININ M225μm/0.25μm 25μm/0.25μmC L =1pFFigure 5.10Low Swing Driver188THE CMOS INVERTER Chapter 5b.What is the voltage swing on the output node as the inputs swing from 0V to 2.5V .Showthe low value and the high value.c.Assume that the inputs IN and IN have zero rise and fall times.Assume a zero skewbetween IN and IN.Determine the low to high propagation delay for charging the outputnode measured from the the 50%point of the input to the 50%point of the output.Assumethat the total load capacitance is 1pF,including the transistor parasitics.d.Assume that,instead of the 1pF load,the low swing driver drives a non-linear capacitor,whose capacitance vs.voltage is plotted pute the energy drawn from the lowsupply for charging up the load capacitor.Ignore the parasitic capacitance of the driver cir-cuit itself.14.The inverter below operates with V DD =0.4V and is composed of |V t |=0.5V devices.Thedevices have identical I 0and n.a.Calculate the switching threshold (V M )of this inverter.b.Calculate V IL and V IH of the inverter.15.Sizing a chain of inverters.a.In order to drive a large capacitance (C L =20pF)from a minimum size gate (with inputcapacitance C i =10fF),you decide to introduce a two-staged buffer as shown in Figure5.12.Assume that the propagation delay of a minimum size inverter is 70ps.Also assumeV DD =0.4VV IN V OUTFigure 5.11Inverter in Weak Inversion RegimeSection 5.1Exercises and Design Problems 189that the input capacitance of a gate is proportional to its size.Determine the sizing of thetwo additional buffer stages that will minimize the propagation delay.b.If you could add any number of stages to achieve the minimum delay,how many stages would you insert?What is the propagation delay in this case?c.Describe the advantages and disadvantages of the methods shown in (a)and (b).d.Determine a closed form expression for the power consumption in the circuit.Consider only gate capacitances in your analysis.What is the power consumption for a supply volt-age of 2.5V and an activity factor of 1?16.[M,None,3.3.5]Consider scaling a CMOS technology by S >1.In order to maintain compat-ibility with existing system components,you decide to use constant voltage scaling.a.In traditional constant voltage scaling,transistor widths scale inversely with S,W ∝1/S.To avoid the power increases associated with constant voltage scaling,however,youdecide to change the scaling factor for W .What should this new scaling factor be to main-tain approximately constant power.Assume long-channel devices (i.e.,neglect velocitysaturation).b.How does delay scale under this new methodology?c.Assuming short-channel devices (i.e.,velocity saturation),how would transistor widthshave to scale to maintain the constant power requirement?1InAdded Buffer StageOUTC L =20pF C i =10fF‘1’is the minimum size inverter.??Figure 5.12Buffer insertion for driving large loads.190THE CMOS INVERTER Chapter5DESIGN PROBLEMUsing the0.25μm CMOS introduced in Chapter2,design a static CMOSinverter that meets the following requirements:1.Matched pull-up and pull-down times(i.e.,t pHL=t pLH).2.t p=5nsec(±0.1nsec).The load capacitance connected to the output is equal to4pF.Notice that thiscapacitance is substantially larger than the internal capacitances of the gate.Determine the W and L of the transistors.To reduce the parasitics,useminimal lengths(L=0.25μm)for all transistors.Verify and optimize the designusing SPICE after proposing a first design using manual -pute also the energy consumed per transition.If you have a layout editor(suchas MAGIC)available,perform the physical design,extract the real circuitparameters,and compare the simulated results with the ones obtained earlier.。

数字电路与系统设计实验

这包括实验方案的正确性、可行性如何？可否进一步优化？有哪些收获体会？有哪些经验教训？有哪些建议？等。
第二章实验基本仪器
数字系统设计实验所需设备有：直流稳压电源，示波器，基于CPLD的数字电路实验系统，万用表，信号源，计算机。
一、直流稳压电源
二、示波器
示波器是一种用来测量电信号波形的电子仪器。用示波器能够观察电信号波形，测量电信号的电压大小，周期信号的频率和周期大小。双踪示波器能够同时观察两路电信号波形。
能块相对集中地排列器件 3.布线顺序 VCC，GND，输入/输出，控制线 4. 仪器检测（电源，示波器，信号源） 5.实验测试、调试与记录
6.撰写实验总结报告
(1)实验内容 (2)实验目的 (3)实验设备 (4)实验方法与手段 (5)实验原理图 (6)实验现象（结果）记录分析 (7)实验结论与体会
（（(四三一）)）、、、实实验实验目验的提内示容
•• １１..注测１意试.掌被T握T测LT器T器L件、件H７的CT４引和Ｌ脚HＳ７C器０和件４引的一脚传个输１特非４性门分。的别传接输地特和十性5。Ｖ２。.掌握万用表的使用方法。
•• •
(２连为输２３特二.接被入)..性将测测、１２３到测电。实试试...被非压六六六验验HH反反反测门值所CC台相相相T器用非的。上器器器器件器门输４件７７７件７的入.４４４７７４输电ＬＨＨ４ＫＨ入压ＳＣＣＨΩＣ００Ｔ端。电Ｃ４４００，旋位Ｔ片片４４转R器０片T一电LR４的个T位一L输非的器个出门电改非端的压变门电传输非的压输出门传作特端的输性。
四、数字电路测试及故障查找、排除
1.数字电路测试
数字电路静态测试指的是给定数字电路若干组静态输入值，测定数字电路的输出值是否正确。

电子设计从零开始_第二版

除了使用图 1-6 中色环与数值关系表判断电阻阻值外，还可以用万用表直接测量电阻，得到阻值的读数。
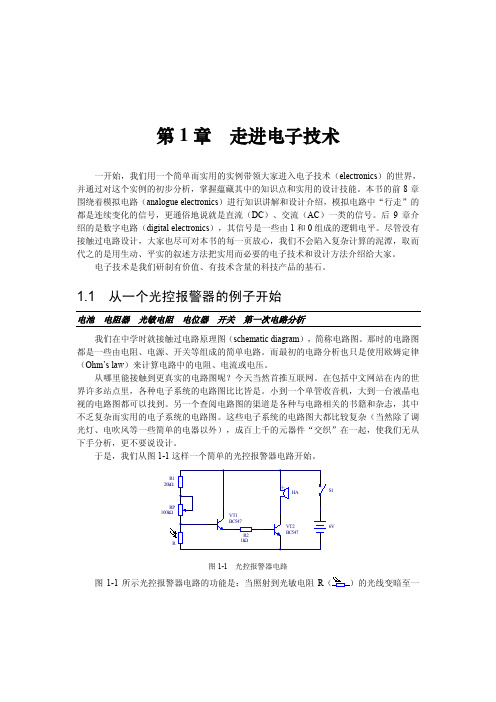
(红色)
(黑色) (黑色)
(棕色)
(金色)
2kΩ，误差±5%
图 1-7 5（色）环电阻阻值
在电路设计选择电阻时应该注意阻值是不可任意选定的，比如标称值为 122Ω 的电阻就不存在。原因是在大部分电路中并不要求极其精确的电阻值，于是为了便于工业上大量生产和使用者在一定范围内选用，EIA（美国电子工业联盟，Electronic Industries Alliance）规定了若干系列的阻值取值基准，其中以 E12 基准和 E24 基准最为常用。
说明电阻可以在电路中改变节点的电压。
在电子市场或网上选购电阻时，至少有 3 个有关参数是需要提供的：一是电阻的阻值，
二是电阻的功率，三是电阻的种类。
1. 电阻的阻值
拿到一支电阻，我们会看到电阻的表面有五颜六色的色环，这不是出于美观而设计的，它标示着电阻的阻值。图 1-6 所示为常用的 5（色）环电阻及颜色所代表的数值。5（色）环电阻使用前 4 个色环标示电阻的阻值，第 5 个色环标示电阻的允许误差。
路的干路电流
I
=
V R
=
VA R1+ R2
=
3V 1kΩ+2kΩ
= 1mA
，则
P
点电压VP
=
IR2
= 1mA × 2kΩ
=
2V
。
从例 1.1 可以看到，节点 A，即电源正极的电压为 3V，通过两个电阻 R1、R2 的“努
力”，节点 P 出现了一个 2V 的电压。这个 2V 电压异于电源电压，是一个人为设计的电压。
图 1-10 大功率电阻

数字集成电路：电路系统与设计(第二版)

数字集成电路：电路系统与设计(第二版)简介《数字集成电路：电路系统与设计(第二版)》是一本介绍数字集成电路的基本原理和设计方法的教材。

本书的内容覆盖了数字电路的基础知识、逻辑门电路、组合逻辑电路、时序逻辑电路、存储器和程序控制电路等方面。

通过学习本书，读者可以了解数字集成电路的概念、设计方法和实际应用。

目录1.数字电路基础知识 1.1 数字电路的基本概念 1.2 二进制系统与数制转换 1.3 逻辑运算与布尔代数2.逻辑门电路 2.1 与门、或门、非门 2.2 与非门、或非门、异或门 2.3 多输入门电路的设计方法3.组合逻辑电路 3.1 组合逻辑电路的基本原理 3.2 组合逻辑电路的设计方法 3.3 编码器和译码器4.时序逻辑电路 4.1 时序逻辑电路的基本原理 4.2 同步时序电路的设计方法 4.3 异步时序电路的设计方法5.存储器电路 5.1 存储器的基本概念 5.2 可读写存储器的设计方法 5.3 只读存储器的设计方法6.程序控制电路 6.1 程序控制电路的基本概念 6.2 程序控制电路的设计方法 6.3 微程序控制器的设计方法内容概述1. 数字电路基础知识本章主要介绍数字电路的基本概念，包括数字电路与模拟电路的区别、数字信号的表示方法以及数制转换等内容。

此外，还介绍了数字电路中常用的逻辑运算和布尔代数的基本原理。

2. 逻辑门电路逻辑门电路是数字电路中的基本组成单元，本章主要介绍了与门、或门、非门以及与非门、或非门、异或门等逻辑门的基本原理和组成。

此外，还介绍了多输入门电路的设计方法，以及逻辑门电路在数字电路设计中的应用。

3. 组合逻辑电路组合逻辑电路是由逻辑门电路组成的，本章主要介绍了组合逻辑电路的基本原理和设计方法。

此外，还介绍了编码器和译码器的原理和应用，以及在数字电路设计中的实际应用场景。

4. 时序逻辑电路时序逻辑电路是在组合逻辑电路的基础上引入了时序元件并进行时序控制的电路。

本章主要介绍了时序逻辑电路的基本原理和设计方法，包括同步时序电路和异步时序电路的设计。

数字集成电路——电路、系统与设计(第二版)Chapter10_timing

Contamination and Propagation Delays
Settle to a final value
Begin to change
Settle to a final value
Begin to change as the last time D begins to change
Settle to a final value as the last time D settles to a final value
Digital Integrated Circuits A Design Perspective
Chapter10: Timing Issues
EE141
Timing Issues
Timing Issues

10.1 Timing Classification
10.2 Synchronous Design
Datapath Structure with Feedback
- Routing the clock so that only negative skew occurs is not feasible
- Design of a low-skew clock network is essential

10.2.5 Clock Distribution Techniques

10.3 Summary
EE141
Timing Issues
Sequencing Methods

Registers

2-Phase Latches
EE141
Timing Issues
Timing Diagrams

数字逻辑电路与系统设计习题答案

第1章习题及解答将下列二进制数转换为等值的十进制数。

（1）（11011）2 （2）（）2（3）（1101101）2 （4）（）2（5）（）2（6）（）2（7）（）2（8）（）2题解：（1）（11011）2 =（27）10 （2）（）2 =（151）10（3）（1101101）2 =（109）10 （4）（）2 =（255）10（5）（）2 =（）10（6）（）2 =（）10（7）（）2=（）10（8）（）2 =（）10将下列二进制数转换为等值的十六进制数和八进制数。

（1）（1010111）2 （2）（1）2（3）（）2 （4）（）2题解：（1）（1010111）2 =（57）16 =（127）8（2）（0）2 =（19A）16 =（632）8（3）（）2 =（）16 =（）8（4）（）2 =（2C.61）16 =（）8将下列十进制数表示为8421BCD码。

（1）（43）10 （2）（）10（3）（）10 （4）（）10题解：（1）（43）10 =（01000011）8421BCD（2）（）10 =（.00010010）8421BCD（3）（）10 =（）8421BCD（4）（）10 =（.0001）8421BCD将下列有符号的十进制数表示成补码形式的有符号二进制数。

（1） +13 （2）−9 （3）+3 （4）−8题解：（1） +13 =（01101）2（2）−9 =（10111）2（3） +3 =（00011）2（4）−8 =（11000）2用真值表证明下列各式相等。

（1）BA+=+B+BBAA（2）()()()=⊕A⊕CACABB（3）()C BA+=+BCA（4）CAB++A=AABC题解：（1）证明BA+=++BABBA（2）证明()()()ACABCBA⊕=⊕（3）证明()C BACBA+=+（4）证明CAB++=AACBA用逻辑代数公式将下列逻辑函数化成最简与或表达式。

（1）D++A=F+BCBCACA（2）()()D++=F+AACCDA（3）()()B++F+=B+DCDBDDA（4）()D++F+=ADCBCBA（5）()C A B C B AC F ⊕++= （6）()()C B B A F ⊕⊕= 题解：（1）BC A D C A BC C A B A F +=+++= （2）()()CD A D CD A C A A F +=+++=（3）()()C B B A D B D A C B D D D B F ++=++++= （4）()D C B A D C B AD C B A F +=+++= （5）()C B AC C A B C B AC F +=⊕++=（6）()()C A BC B A C B B A F ++=⊕⊕=或C A C B AB ++= 用卡诺图将下列逻辑函数化成最简与或表达式。

数字集成电路--电路、系统与设计(第二版)复习资料

第一章数字集成电路介绍第一个晶体管，Bell 实验室，1947第一个集成电路，Jack Kilby ，德州仪器，1958 摩尔定律：1965年，Gordon Moore 预言单个芯片上晶体管的数目每18到24个月翻一番。

(随时间呈指数增长)抽象层次：器件、电路、门、功能模块和系统抽象即在每一个设计层次上，一个复杂模块的内部细节可以被抽象化并用一个黑匣子或模型来代替。

这一模型含有用来在下一层次上处理这一模块所需要的所有信息。

固定成本（非重复性费用）与销售量无关；设计所花费的时间和人工；受设计复杂性、设计技术难度以及设计人员产出率的影响；对于小批量产品，起主导作用。

可变成本（重复性费用）与产品的产量成正比；直接用于制造产品的费用；包括产品所用部件的成本、组装费用以及测试费用。

每个集成电路的成本=每个集成电路的可变成本+固定成本/产量。

可变成本=（芯片成本+芯片测试成本+封装成本）/最终测试的成品率。

一个门对噪声的灵敏度是由噪声容限NM L （低电平噪声容限）和NM H （高电平噪声容限）来度量的。

为使一个数字电路能工作，噪声容限应当大于零，并且越大越好。

NM H = V OH - V IH NM L = V IL - V OL 再生性保证一个受干扰的信号在通过若干逻辑级后逐渐收敛回到额定电平中的一个。

一个门的VTC 应当具有一个增益绝对值大于1的过渡区(即不确定区)，该过渡区以两个有效的区域为界，合法区域的增益应当小于1。

理想数字门特性：在过渡区有无限大的增益；门的阈值位于逻辑摆幅的中点；高电平和低电平噪声容限均等于这一摆幅的一半；输入和输出阻抗分别为无穷大和零。

传播延时、上升和下降时间的定义传播延时tp 定义了它对输入端信号变化的响应有多快。

它表示一个信号通过一个门时所经历的延时，定义为输入和输出波形的50%翻转点之间的时间。

上升和下降时间定义为在波形的10%和90%之间。

对于给定的工艺和门的拓扑结构，功耗和延时的乘积一般为一常数。

数字电路第一章数制和码制

（ 0 1 1 0 1 0 1 0 . 0 1 )2
0
0
（2）八进制数转换为二进制数：将每位八进制数用3位二进制数表示。
＝ (152.2)8
(
3
7
4 .
2
6)8
= （ 011 111 100 . 010 110）2
十六－二转换
二进制数与十六进制数的相互转换，按照每4位二进制数对应于一位十六进制数进行转换。
( N )R
i m
a R
i
n 1
i
1 原码
又称"符号+数值表示", 对于正数, 符
号位为0, 对于负数、符号位为1, 其余各位表示数值部分。
例： N1 = +10011
[ N1]原= 010011
N2 = – 01010
[N2]原= 101010
原码表示的特点: 真值0有两种原码表示形式, 即 [ +0]原= 00…0 [– 0]原= 1 0…0
求[ N1 +N2]原，绝对值相减，有
[ N1 +N2]原＝01000
二、反码运算
[ N1 +N2]反＝ [ N1]反+ [ N2]反
[ N1 －N2]反＝ [ N1]反+ [－ N2]反当符号位有进位时，应在结果的最低位再加"1".
例： N1 =－0011，N2 = 1011求[ N1 +N2]反和 [ N1 －N2]反。
N10
i m
K i 10i
n 1
式中Kｉ为基数10的ｉ次幂的系数，它可为0～9 中的任一个数字。
如 .58)10 2 102 3 101 4 100 5 101 (234 102 8