The number of maximum independent sets in graphs

合集下载

运筹学英汉词汇ABC

运筹学英汉词汇(0,1) normalized ――0-1规范化Aactivity ――工序additivity――可加性adjacency matrix――邻接矩阵adjacent――邻接aligned game――结盟对策analytic functional equation――分析函数方程approximation method――近似法arc ――弧artificial constraint technique ――人工约束法artificial variable――人工变量augmenting path――增广路avoid cycle method ――避圈法Bbackward algorithm――后向算法balanced transportation problem――产销平衡运输问题basic feasible solution ――基本可行解basic matrix――基阵basic solution ――基本解basic variable ――基变量basic ――基basis iteration ――换基迭代Bayes decision――贝叶斯决策big M method ――大M 法binary integer programming ――0-1整数规划binary operation――二元运算binary relation――二元关系binary tree――二元树binomial distribution――二项分布bipartite graph――二部图birth and death process――生灭过程Bland rule ――布兰德法则branch node――分支点branch――树枝bridge――桥busy period――忙期Ccapacity of system――系统容量capacity――容量Cartesian product――笛卡儿积chain――链characteristic function――特征函数chord――弦circuit――回路coalition structure――联盟结构coalition――联盟combination me――组合法complement of a graph――补图complement of a set――补集complementary of characteristic function――特征函数的互补性complementary slackness condition ――互补松弛条件complementary slackness property――互补松弛性complete bipartite graph――完全二部图complete graph――完全图completely undeterministic decision――完全不确定型决策complexity――计算复杂性congruence method――同余法connected component――连通分支connected graph――连通图connected graph――连通图constraint condition――约束条件constraint function ――约束函数constraint matrix――约束矩阵constraint method――约束法constraint ――约束continuous game――连续对策convex combination――凸组合convex polyhedron ――凸多面体convex set――凸集core――核心corner-point ――顶点（角点）cost coefficient――费用系数cost function――费用函数cost――费用criterion ; test number――检验数critical activity ――关键工序critical path method ――关键路径法（CMP ）critical path scheduling ――关键路径cross job ――交叉作业curse of dimensionality――维数灾customer resource――顾客源customer――顾客cut magnitude ――截量cut set ――截集cut vertex――割点cutting plane method ――割平面法cycle ――回路cycling ――循环Ddecision fork――决策结点decision maker决――策者decision process of unfixed step number――不定期决策过程decision process――决策过程decision space――决策空间decision variable――决策变量decision决－－策decomposition algorithm――分解算法degenerate basic feasible solution ――退化基本可行解degree――度demand――需求deterministic inventory model――确定贮存模型deterministic type decision――确定型决策diagram method ――图解法dictionary ordered method ――字典序法differential game――微分对策digraph――有向图directed graph――有向图directed tree――有向树disconnected graph――非连通图distance――距离domain――定义域dominate――优超domination of strategies――策略的优超关系domination――优超关系dominion――优超域dual graph――对偶图Dual problem――对偶问题dual simplex algorithm ――对偶单纯形算法dual simplex method――对偶单纯形法dummy activity――虚工序dynamic game――动态对策dynamic programming――动态规划Eearliest finish time――最早可能完工时间earliest start time――最早可能开工时间economic ordering quantity formula――经济定购批量公式edge ――边effective set――有效集efficient solution――有效解efficient variable――有效变量elementary circuit――初级回路elementary path――初级通路elementary ――初等的element――元素empty set――空集entering basic variable ――进基变量equally liability method――等可能性方法equilibrium point――平衡点equipment replacement problem――设备更新问题equipment replacing problem――设备更新问题equivalence relation――等价关系equivalence――等价Erlang distribution――爱尔朗分布Euler circuit――欧拉回路Euler formula――欧拉公式Euler graph――欧拉图Euler path――欧拉通路event――事项expected value criterion――期望值准则expected value of queue length――平均排队长expected value of sojourn time――平均逗留时间expected value of team length――平均队长expected value of waiting time――平均等待时间exponential distribution――指数分布external stability――外部稳定性Ffeasible basis ――可行基feasible flow――可行流feasible point――可行点feasible region ――可行域feasible set in decision space――决策空间上的可行集feasible solution――可行解final fork――结局结点final solution――最终解finite set――有限集合flow――流following activity ――紧后工序forest――森林forward algorithm――前向算法free variable ――自由变量function iterative method――函数迭代法functional basic equation――基本函数方程function――函数fundamental circuit――基本回路fundamental cut-set――基本割集fundamental system of cut-sets――基本割集系统fundamental system of cut-sets――基本回路系统Ggame phenomenon――对策现象game theory――对策论game――对策generator――生成元geometric distribution――几何分布goal programming――目标规划graph theory――图论graph――图HHamilton circuit――哈密顿回路Hamilton graph――哈密顿图Hamilton path――哈密顿通路Hasse diagram――哈斯图hitchock method ――表上作业法hybrid method――混合法Iideal point――理想点idle period――闲期implicit enumeration method――隐枚举法in equilibrium――平衡incidence matrix――关联矩阵incident――关联indegree――入度indifference curve――无差异曲线indifference surface――无差异曲面induced subgraph――导出子图infinite set――无限集合initial basic feasible solution ――初始基本可行解initial basis ――初始基input process――输入过程Integer programming ――整数规划inventory policy―v存贮策略inventory problem―v货物存储问题inverse order method――逆序解法inverse transition method――逆转换法isolated vertex――孤立点isomorphism――同构Kkernel――核knapsack problem ――背包问题Llabeling method ――标号法latest finish time――最迟必须完工时间leaf――树叶least core――最小核心least element――最小元least spanning tree――最小生成树leaving basic variable ――出基变量lexicographic order――字典序lexicographic rule――字典序lexicographically positive――按字典序正linear multiobjective programming――线性多目标规划Linear Programming Model――线性规划模型Linear Programming――线性规划local noninferior solution――局部非劣解loop method――闭回路loop――圈loop――自环（环）loss system――损失制Mmarginal rate of substitution――边际替代率Marquart decision process――马尔可夫决策过程matching problem――匹配问题matching――匹配mathematical programming――数学规划matrix form ――矩阵形式matrix game――矩阵对策maximum element――最大元maximum flow――最大流maximum matching――最大匹配middle square method――平方取中法minimal regret value method――最小后悔值法minimum-cost flow――最小费用流mixed expansion――混合扩充mixed integer programming ――混合整数规划mixed Integer programming――混合整数规划mixed Integer ――混合整数规划mixed situation――混合局势mixed strategy set――混合策略集mixed strategy――混合策略mixed system――混合制most likely estimate――最可能时间multigraph――多重图multiobjective programming――多目标规划multiobjective simplex algorithm――多目标单纯形算法multiple optimal solutions ――多个最优解multistage decision problem――多阶段决策问题multistep decision process――多阶段决策过程Nn- person cooperative game ――n人合作对策n- person noncooperative game――n人非合作对策n probability distribution of customer arrive――顾客到达的n 概率分布natural state――自然状态nature state probability――自然状态概率negative deviational variables――负偏差变量negative exponential distribution――负指数分布network――网络newsboy problem――报童问题no solutions ――无解node――节点non-aligned game――不结盟对策nonbasic variable ――非基变量nondegenerate basic feasible solution――非退化基本可行解nondominated solution――非优超解noninferior set――非劣集noninferior solution――非劣解nonnegative constrains ――非负约束non-zero-sum game――非零和对策normal distribution――正态分布northwest corner method ――西北角法n-person game――多人对策nucleolus――核仁null graph――零图Oobjective function ――目标函数objective( indicator) function――指标函数one estimate approach――三时估计法operational index――运行指标operation――运算optimal basis ――最优基optimal criterion ――最优准则optimal solution ――最优解optimal strategy――最优策略optimal value function――最优值函数optimistic coefficient method――乐观系数法optimistic estimate――最乐观时间optimistic method――乐观法optimum binary tree――最优二元树optimum service rate――最优服务率optional plan――可供选择的方案order method――顺序解法ordered forest――有序森林ordered tree――有序树outdegree――出度outweigh――胜过Ppacking problem ――装箱问题parallel job――平行作业partition problem――分解问题partition――划分path――路path――通路pay-off function――支付函数payoff matrix――支付矩阵payoff――支付pendant edge――悬挂边pendant vertex――悬挂点pessimistic estimate――最悲观时间pessimistic method――悲观法pivot number ――主元plan branch――方案分支plane graph――平面图plant location problem――工厂选址问题player――局中人Poisson distribution――泊松分布Poisson process――泊松流policy――策略polynomial algorithm――多项式算法positive deviational variables――正偏差变量posterior――后验分析potential method ――位势法preceding activity ――紧前工序prediction posterior analysis――预验分析prefix code――前级码price coefficient vector ――价格系数向量primal problem――原问题principal of duality ――对偶原理principle of optimality――最优性原理prior analysis――先验分析prisoner’s dilemma――囚徒困境probability branch――概率分支production scheduling problem――生产计划program evaluation and review technique――计划评审技术(PERT) proof――证明proper noninferior solution――真非劣解pseudo-random number――伪随机数pure integer programming ――纯整数规划pure strategy――纯策略Qqueue discipline――排队规则queue length――排队长queuing theory――排队论Rrandom number――随机数random strategy――随机策略reachability matrix――可达矩阵reachability――可达性regular graph――正则图regular point――正则点regular solution――正则解regular tree――正则树relation――关系replenish――补充resource vector ――资源向量revised simplex method――修正单纯型法risk type decision――风险型决策rooted tree――根树root――树根Ssaddle point――鞍点saturated arc ――饱和弧scheduling (sequencing) problem――排序问题screening method――舍取法sensitivity analysis ――灵敏度分析server――服务台set of admissible decisions(policies) ――允许决策集合set of admissible states――允许状态集合set theory――集合论set――集合shadow price ――影子价格shortest path problem――最短路线问题shortest path――最短路径simple circuit――简单回路simple graph――简单图simple path――简单通路Simplex method of goal programming――目标规划单纯形法Simplex method ――单纯形法Simplex tableau――单纯形表single slack time ――单时差situation――局势situation――局势slack variable ――松弛变量sojourn time――逗留时间spanning graph――支撑子图spanning tree――支撑树spanning tree――生成树stable set――稳定集stage indicator――阶段指标stage variable――阶段变量stage――阶段standard form――标准型state fork――状态结点state of system――系统状态state transition equation――状态转移方程state transition――状态转移state variable――状态变量state――状态static game――静态对策station equilibrium state――统计平衡状态stationary input――平稳输入steady state――稳态stochastic decision process――随机性决策过程stochastic inventory method――随机贮存模型stochastic simulation――随机模拟strategic equivalence――策略等价strategic variable, decision variable ――决策变量strategy (policy) ――策略strategy set――策略集strong duality property ――强对偶性strong ε-core――强ε-核心strongly connected component――强连通分支strongly connected graph――强连通图structure variable ――结构变量subgraph――子图sub-policy――子策略subset――子集subtree――子树surplus variable ――剩余变量surrogate worth trade-off method――代替价值交换法symmetry property ――对称性system reliability problem――系统可靠性问题Tteam length――队长tear cycle method――破圈法technique coefficient vector ――技术系数矩阵test number of cell ――空格检验数the branch-and-bound technique ――分支定界法the fixed-charge problem ――固定费用问题three estimate approach一―时估计法total slack time――总时差traffic intensity――服务强度transportation problem ――运输问题traveling salesman problem――旅行售货员问题tree――树trivial graph――平凡图two person finite zero-sum game二人有限零和对策two-person game――二人对策two-phase simplex method ――两阶段单纯形法Uunbalanced transportation problem ――产销不平衡运输问题unbounded ――无界undirected graph――无向图uniform distribution――均匀分布unilaterally connected component――单向连通分支unilaterally connected graph――单向连通图union of sets――并集utility function――效用函数Vvertex――顶点voting game――投票对策Wwaiting system――等待制waiting time――等待时间weak duality property ――弱对偶性weak noninferior set――弱非劣集weak noninferior solution――弱非劣解weakly connected component――弱连通分支weakly connected graph――弱连通图weighed graph ――赋权图weighted graph――带权图weighting method――加权法win expectation――收益期望值Zzero flow――零流zero-sum game――零和对策zero-sum two person infinite game――二人无限零和对策。

数学专业英语2-10翻译

Although dependence and independence are properties of sets of elements, we also apply these terms to the elements themselves. For example, the elements in an independent set are called independent elements.虽然相关和无关是元素集的属性，我们也适用于这些元素本身。

例如，在一个独立设定的元素被称为独立元素。

If s is finite set, the foregoing definition agrees with that given in Chapter 8 for the space n V . However, the present definition is not restricted to finite sets.如果S 是有限集，同意上述定义与第8章中给出的空间n V ，然而，目前的定义不局限于有限集。

If a subset T of a set S is dependent, then S itself is dependent. This is logically equivalent to the statement that every subset of an independent set is independent.如果集合S 的子集T 是相关的，然后S 本身是相关的，这在逻辑上相当于每一个独立设置的子集是独立的语句。

If one element in S is a scalar multiple of another, then S is dependent. 如果S 中的一个元素是另一个集中的多个标量的，则S 是相关的。

If S ∈0,then S is dependent. 若S ∈0，则 S 是相关的。

偏最小二乘法(pls)简介(Partial least squares (PLS) Introduction)

---------------------------------------------------------------最新资料推荐------------------------------------------------------偏最小二乘法(pls)简介（Partial least squares(PLS) Introduction）偏最小二乘法(pls)简介（Partial least squares (PLS) Introduction）偏最小二乘法(pls)简介（Partial least squares (PLS) Introduction） Partial least squares (PLS). The news about | | | | | Software Research Report training | knowledge sharing | customer list | Forum Partial least squares (PLS). Author: CIC reading: 14122 times the release date: 2004-12-30 Brief introduction The partial least squares method is a new type of multivariate statistical data analysis method, it was founded in 1983 by Wood (S.Wold) and Abano (C.Albano), is proposed for the first time. In recent decades, it has been developed rapidly in theory, method and application. The partial least squares method For a long time, the boundaries between the methods of model type and understanding of the share is very clear. The partial least squares rule them organically, in an algorithm, can simultaneously achieve regression (multivariate linear regression), simplify the structure of the data (principal component analysis) and the correlation between the two sets of variables analysis (canonical correlation analysis). This is a leap in the multivariate1/ 9statistical analysis of data. The importance of partial least squares method in statistical applications are reflected in the following aspects: Partial least squares regression modeling method is a multi variable for multiple variables. The partial least squares method can solve many previous ordinary regression can not solve the problem. The partial least squares method is called the second generation regression method, because it can realize the comprehensive application of various data analysis methods. The main purpose of principal component regression is to extract relevant information hidden in the matrix X, and then used to predict the value of the variable Y. This approach can ensure that we use only those independent variables, the noise will be eliminated, so as to improve the quality of the prediction model. However, the principal component regression still has some defects, when the correlation between some useful variables is very small, we are in the selection of the main components is easy to put them out, make the reliability prediction model for final decline, if we choose for each component, it is too difficult. Partial least squares regression can solve this problem. It adopts the way of decomposition of the variables X and Y, while extracting components from the variables X and---------------------------------------------------------------最新资料推荐------------------------------------------------------Y (usually called factor), then the factor according to the correlation between them in order from large to small. Now, we want to build a model, as long as we choose several factors involved in modeling it The basic concept Partial least squares regression is an expansion of the multivariate linear regression model, in its simplest form, only a linear model to describe the relationship between the independent variables and Y variables X: Y = B0 + b1X1 + b2X2 + bpXp +... In the equation, B0 is intercept, the value of Bi is 1 to the data points of regression coefficient P. For example, we can think of a person’s weight is a function of his height, gender, and from their respective sample points to estimate the regression coefficient, we can generally predict the weight of someone from the measured height and gender. Many of the methods of data analysis, data describing the biggest problem accurately and make a reasonable forecast of new observational data. The multiple linear regression model to deal with more complex data analysis problems, extended some other algorithms, like discriminant analysis, principal component regression, correlation analysis and so on, are multivariate statistical method with multiple linear regression model based. These3/ 9multivariate statistical methods there are two important characteristics, namely the data binding: The factor variable X and variable Y must be extracted from the X’X and Y’Y matrix, these factors cannot be said at the same time correlation between the variables X and Y. The number of prediction equation can never be more than the variable Y with variable X. Partial least squares regression from multiple linear regression and its expansion did not need these data constraints. In the partial least squares regression, prediction equation by the factor extracted from the matrix Y’XX’Y to describe; in order to be more representative, the maximum number of the number of prediction equations extracted may be greater than the variable X and Y. In short, partial least squares regression may be all multivariate calibration methods for the least variable constraint method, this flexibility makes it suitable for many occasions in the traditional multivariate calibration method is not applicable, for example, some observational data less than when the number of predictor variables. Moreover, partial least squares regression can be used as an exploratory analysis tool, before using the traditional linear regression model, first on the number of variables required for appropriate prediction and---------------------------------------------------------------最新资料推荐------------------------------------------------------ removal of noise. Therefore, the partial least squares regression is widely used in many fields of modeling, such as chemical, medicine, economics, psychology and pharmaceutical science and so on, especially it can according to arbitrarily set the variable this advantage is more prominent. In chemometrics, partial least squares regression has been used as a standard multivariate modeling tool. The calculation process The basic model As a method of multiple linear regression, partial least squares regression main purpose is to establish a linear model: Y=XB+E, where Y is the response matrix with m variables, n points, X is the prediction matrix with P variables, n sample, B regression coefficient matrix, E noise the calibration model, which has the same dimension with Y. In general, the variables X and Y was then used to calculate the standard, i.e. minus their average value and standard deviation divided by the. Partial least squares regression and principal component regression, using factor score as the original prediction variable as a linear combination of the basis, so used to establish prediction model between factor scores must be linearly independent. For example, if we have a set of response variables (Y matrix) and now a large number5/ 9of predictor variables X (matrix), some serious variable linear correlation, we use factor extracted from this set of data extraction method is used to calculate the factor, factor score matrix T=XW, then calculate the weight matrix W right then, a linear regression model: Y=TQ+E, where Q is the regression coefficient matrix of T matrix, E matrix error. Once the Q is calculated after the previous equation is equivalent to the Y=XB+E, the B=WQ, it can be directly used as predictive regression model. The difference of partial least squares regression and principal component regression in different extraction methods of factor score in short weight matrix W principal component regression generated reflects the prediction covariance between variables X, partial least squares W regression weight matrix generated reflects the covariance between the predictor variables and the response variable Y X. In the model, partial least squares regression produces weight matrix W PXC, column vector matrix for W score matrix T column vector calculation variable X nxc. The calculation of these weights the response covariance between the corresponding factor score reaches maximum constant. Ordinary least squares regression matrix Q regression on T when calculating Y, the matrix Y load factor (or weight), are used---------------------------------------------------------------最新资料推荐------------------------------------------------------to establish the regression equation: Y=TQ+E. Once calculated Q, we can get the equation: Y=XB+E, B=WQ, the final prediction model is built up. The nonlinear iterative partial least squares method For a standard algorithm for computing partial least squares regression is a nonlinear iterative partial least squares (NIPALS), there are many variables in this algorithm, some have been standardized, some are not. The following algorithm is considered to be one of the most effective method in the nonlinear iterative partial least squares. On C, A0=X’Y and h=1..., M0=X’X, C0=I, C of known variables. Calculation of QH, the principal eigenvectors of Ah’Ah. Wh=GhAhqh, wh=wh/||wh||, and wh as the column vector of W. Ph=Mhwh, ch=wh’Mhwh, ph=ph/ch, and pH as the column vect or of P. Qh=Ah’wh/ch, and QH as the column vector of Q. Ah+1=Ah - chphqh’, Bh+1=Mh - chphph’ Ch+1=Ch - whph’ Factor score matrix T can be calculated: T=XW, partial least squares regression coefficient B can be calculated by the formula B=WQ. SIMPLS algorithm There is a method to estimate the regression component of partial least squares, called SIMPLS algorithm. On C, A0=X’Y and h=1..., M0=X’X, C0=I, C of known variables. Calculation of QH, the principal eigenvectors of Ah’Ah.7/ 9Wh=Ahqh, ch=wh’Mhwh, wh=wh/sqrt (CH), and wh as the column vector of W. Ph=Mhwh, and pH as the column vector of P. Qh=Ah’wh, and QH as the column vector of Q. Vh=Chph, vh=vh/||vh|| Ch+1=Ch - vhvh’, Mh+1=Mh - phph’ Ah+1=ChAh The same as NIPALS, SIMPLS T T=XW calculated by the formula, B formula by B=WQ’calculation. Related literature Xu Lu, chemometrics methods, Science Press, Beijing, 1995. Wang Huiwen, the partial least squares regression method and application, National Science and Technology Press, Beijing, 1996. Chin, W. W., and Newsted, P. R. Structural Equation (1999). Modeling analysis with Small Samples Using Partial Least Squares. In Rick Hoyle (Ed.), Statistical Strategies for Small Sample Research, Sage Publications. Chin, W. W. (1998) The partial least squares approach for. Structural equation modelling. In George A. Marcoulides (Ed.), Modern Methods for Business Research, Lawrence Erlbaum Associates. Barclay, D., C. Higgins and R. Thompson The Partial (1995). Least Squares (PLS) Approach to Causal Modeling: Personal Computer Adoption and Use as an Illustration. Technology Studies, Volume 2, issue 2, 285-309. Chin, W. W. (1995). Partial Least Squares Is To LISREL As 主成分分析是常见的因素分析。

数学英语如何用英语表达数学术语

absolute value 绝对值acceptable region 接受域additivity 可加性alternative hypothesis 对立假设analysis of covariance 协方差分析analysis of variance 方差分析arithmetic mean 算术平均值association 相关性assumption checking 假设检验availability 有效度band 带宽bar chart 条形图beta-distribution 贝塔分布between groups 组间的binomial distribution 二项分布binomial test 二项检验center of gravity 重心central tendency 中心趋势hi-square distribution 卡方分布chi-square test 卡方检验classify 分类cluster analysis 聚类分析coefficient 系数coefficient of correlation 相关系数collinearity 共线性components 构成，分量compound 复合的confidence interval 置信区间consistency 一致性continuous variable 连续变量control charts 控制图correlation 相关covariance 协方差covariance matrix 协方差矩阵critical point 临界点critical value 临界值cross tab 列联表cubic term 三次项cumulative distribution function 累加分布函数curve estimation 曲线估计default 默认的deleted residual 剔除残差density function 密度函数dependent variable 因变量design of experiment 试验设计df.(degree of freedom) 自由度diagnostic 诊断discrete variable 离散变量discriminant function 判别函数discriminatory analysis 判别分析D-optimal design D-优化设计effects of interaction 交互效应eigenvalue 特征值equal size 等含量estimation of parameters 参数估计estimations 估计量exact value 精确值expected value 期望值exponential指数的exponential distribution 指数分布extreme value 极值factor analysis 因子分析factor score 因子得分factorial designs 析因设计factorial experiment 析因试验fitted line 拟合线fitted value 拟合值fixed variable 固定变量fractional factorial design 部分析因设计F-test F检验full factorial design 完全析因设计gamma distribution 伽玛分布geometric mean 几何均值harmonic mean 调和均值heterogeneity 不齐性histogram 直方图homogeneity 齐性homogeneity of variance 方差齐性hypothesis test 假设检验independence独立independent variable 自变量independent-samples 独立样本index of correlation 相关指数interclass correlation 组内相关interval estimate 区间估计inverse 倒数的iterate 迭代kurtosis 峰度large sample problem 大样本问题least-significant difference 最小显著差数least-square estimation 最小二乘估计least-square method 最小二乘法level of significance 显著性水平leverage value 中心化杠杆值life test 寿命试验likelihood function 似然函数likelihood ratio test 似然比检验linear estimator 线性估计linear model 线性模型linear regression 线性回归linear relation 线性关系linear term 线性项logarithmic 对数的logarithms 对数lost function 损失函数main effect 主效应matrix 矩阵maximum 最大值maximum likelihood estimation 极大似然估计mean squared deviation(MSD) 均方差mean sum of square 均方和measure 衡量media 中位数M-estimator M估计minimum 最小值missing values 缺失值mixed model 混合模型mode 众数Monte Carle method 蒙特卡罗法moving average移动平均值multicollinearity 多元共线性multiple comparison 多重比较multiple correlation 多重相关multiple correlation coefficient 复相关系数multiple correlation coefficient 多元相关系数multiple regression analysis 多元回归分析multiple regression equation 多元回归方程multiple response 多响应multivariate analysis 多元分析negative nonadditively 不可加性nonlinear 非线性nonlinear regression 非线性回归noparametric tests 非参数检验normal distribution 正态分布null hypothesis 零假设number of cases 个案数one-sample 单样本one-tailed test 单侧检验one-way ANOV A 单向方差分析one-way classification 单向分类optimal 优化的optimum allocation 最优配制order statistics 次序统计量origin 原点orthogonal 正交的outliers 异常值paired observations 成对观测数据paired-sample 成对样本parameter estimation 参数估计partial correlation 偏相关partial correlation coefficient 偏相关系数partial regression coefficient 偏回归系percentiles 百分位数pie chart 饼图point estimate 点估计poisson distribution 泊松分布polynomial curve 多项式曲线polynomial regression 多项式回归polynomials 多项式positive relationship 正相关power 幂P-P plot P-P概率图predicted value 预测值prediction intervals 预测区间principal component analysis 主成分分析proability 概率probability density function 概率密度函数quadratic 二次的Q-Q plot Q-Q概率图quadratic term 二次项quality control 质量控制quantitative 数量的，度量quartiles 四分位数random sampling 随机取样random seed 随机数种子random variable 随机变量randomization 随机化range 极差rank correlation 秩相关rank statistic 秩统计量regression analysis 回归分析regression coefficient 回归系数regression line 回归线rejection region 拒绝域residual 残差residual sum of squares 剩余平方和risk function 风险函数robustness 稳健性root mean square 标准差row 行run test 游程检验sample size 样本容量sample space 样本空间sampling 取样sampling inspection 抽样检验scatter chart 散点图S-curve S形曲线sets 集合sign test 符号检验significance level 显著性水平significance testing 显著性检验significant digits 有效数字skewed distribution 偏态分布small sample problem 小样本问题sort 排序sources of variation 方差来源ion 标准离差standard error of mean 均值的标准误差statistical quality control 统计质量控制std. residual 标准残差stepwise regression analysis 逐步回归strong assumption 强假设stud. deleted residual 学生化剔除残差stud. residual 学生化残差subsamples 次级样本sufficient statistic 充分统计量sum of squares 平方和t-distribution t分布test criterion 检验判据test for linearity 线性检验test of goodness of fit 拟合优度检验test of homogeneity 齐性检验test of independence 独立性检验test rules 检验法则test statistics 检验统计量testing function 检验函数time series 时间序列tolerance limits 容许限trimmed mean 截尾均值true value 真值t-test t检验two-tailed test 双侧检验unbiased estimation 无偏估计unbiasedness 无偏性uniform distribution 均匀分布value of estimator 估计值variance 方差variance components 方差分量variance ratio 方差比weighted average 加权平均值within groups 组内的Z score Z分数active constraint 活动约束active set method 活动集法analytic gradient 解析梯度approximate 近似arbitrary 强制性的argument 变量attainment factor 达到因子bandwidth 带宽be equivalent to 等价于best-fit 最佳拟合coefficient 系数complex-value 复数值component 分量constrained 有约束的constraint function 约束函数converge 收敛cubic polynomial interpolation method 三次多项式插值法curve-fitting 曲线拟合data-fitting 数据拟diagonal 对角的direct search method 直接搜索法direction of search 搜索方向eigenvalue 特征值empty matrix 空矩阵exceeded 溢出的feasible solution 可行解finite-difference 有限差分first-order 一阶Gauss-Newton method 高斯-牛顿法goal attainment problem 目标达到问题gradient method 梯度法handle 句柄Hessian matrix 海色矩阵independent variables 独立变量inequality 不等式infeasibility 不可行性initial feasible solution 初始可行解initialize 初始化invoke 激活iteration 迭代Jacobian 雅可比矩阵Lagrange multiplier 拉格朗日乘子large-scale 大型的least square 最小二乘least squares sense 最小二乘意义上的Levenberg-Marquardt method 列文伯格-马夸尔特法line search 一维搜索linear equality constraints 线性等式约束linear programming problem 线性规划问题local solution 局部解medium-scale 中型的mixed quadratic and cubic polynomial interpolation and extrapolationmethod 混合二次、三次多项式内插、外插法multi objective 多目标的norm 范数observed data 测量数据optimization routine 优化过程optimizer 求解器over-determined system 超定系统partial derivatives 偏导数polynomial interpolation method 多项式插值法quadrati二次的quadratic interpolation method 二次内插法quadratic programming 二次规划real-value 实数值residuals 残差robust 稳健的robustness 稳健性，鲁棒性scalar 标量semi-infinitely problem 半无限问题Sequential Quadratic Programming method 序列二次规划法simplex search method 单纯形法sparse matrix 稀疏矩阵sparsity pattern 稀疏模式sparsity structure 稀疏结构starting point 初始点step length 步长subspace trust region method 子空间置信域法symmetric matrix 对称矩阵termination message 终止信息termination tolerance 终止容限the exit condition 退出条件the method of steepest descent 最速下降法transpose 转置unconstrained 无约束的under-determined system 负定系统weighting matrix 加权矩阵approximation 逼近a spline in b-form/b-spline b样条 a spline of polynomial piece /ppform spline 分段多项式样条bivariate spline function 二元样条函数break/breaks 断点coefficient/coefficients 系数cubic interpolation 三次插值/三次内插cubic polynomial 三次多项式cubic smoothing spline 三次平滑样条cubic spline 三次样条cubic spline interpolation 三次样条插值/三次样条内插curve 曲线degree of freedom 自由度end conditions 约束条件input argument 输入参数interpolation 插值/内插interval 取值区间knot/knots 节点least-squares approximation 最小二乘拟合multiplicity 重次multivariate function 多元函数optional argument 可选参数output argument 输出参数point/points 数据点rational spline 有理样条rounding error 舍入误差（相对误差）sequence 数列（数组spline approximation 样条逼近/样条拟合spline function 样条函数spline curve 样条曲线spline interpolation 样条插值/样条内插spline surface 样条曲面smoothing spline 平滑样条tolerance 允许精度univariate function 一元函数absolute error 绝对误差absolute tolerance 绝对容限adaptive mesh 适应性网格boundary condition 边界条件contour plot 等值线图coordinate 坐标系decomposed geometry matrix 分解几何矩阵diagonal matrix 对角矩阵Dirichlet boundary conditions 边界条件eigenvalue 特征值elliptic 椭圆形的error estimate 误差估计exact solution 精确解generalized Neumann boundary condition 推广的Neumann边界条件geometry description matrix 几何描述矩阵geometry matrix 几何矩阵graphical user interface（GUI）图形用户界面hyperbolic 双曲线的initial mesh 初始网格jiggle 微调Lagrange multipliers 拉格朗日乘子Laplace equation 拉普拉斯方程linear interpolation 线性插值machine precision 机器精度mixed boundary condition 混合边界条件Neuman boundary condition Neuman边界条件node point 节点nonlinear solver 非线性求解器normal vector 法向量Parabolic 抛物线型的partial differential equation 偏微分方程plane strain 平面应变plane stress 平面应力Poisson's equation 泊松方程polygon 多边形positive definite 正定refined triangular mesh 加密的三角形网格relative tolerance 相对容限relative tolerance 相对容限residual norm 残差范数singular 奇异的postulate假定, 基本条件, 基本原理,要求, 假定,要求conic, conical圆锥的；圆锥形的ellipse椭圆, 椭圆形ellipt hyperbolic 双曲线的parabolic用寓言表达的: 抛物线的，像抛物线的algebraic代数的, 关于代数学的mineralogy 矿物学axiom公理collinear在同一直线上的同线的convex 凸出的；凸面的triangle三角形, 三人一组, 三角关系parallelogram平行四边形straight angle平角right angle 直角acute angle锐角obtuse angle钝角reflex angle优角rectilinear直线的；由直线组成的；循直线进行的isosceles triangle等腰三角形equilateral triangle等边三角形right triangle n. 直角三角形obtuse triangle钝角三角形acute triangle锐角三角形equiangular triangle正三角形,等角三角形hypotenuse（直角三角形的）斜边infinitesimal 无穷小的, 极小的, 无限小的calculus 微积分学, 结石inscribe 记下polygon多角形, 多边形curvilinear曲线的, 由曲线组成的intuition 直觉, 直觉的知识integral积分, 完整, 部分defective有缺陷的, (智商或行为有)欠缺的differential coefficient 微分系数irrational numbers无理数domain 定义域contradiction 矛盾continuous variable 连续变量;［连续变数］variation 变分, 变化independent variable 自变量dependent variable 应变量rectangular coordinate 直角坐标abscissa〈数〉横坐标ordinate纵线, 纵座标differential 微分的,微分(differentiation)Integral 积分, 完整, 部分(integration) trigonometry 三角法exponential 指数的, 幂数的logarithm 对数derivative导数；微商tangent 切线正切definite integral 定积分culminate 达到顶点differential equation 微分方程extreme value 极值multiple integral 多重积分functional analysis 泛函分析cardinal number 基数（如：1, 2, 3, ... 有别于序数）denumerable可数的aggregate 合计, 总计, 集合体，合计的, 集合的, 聚合的，聚集, 集合, 合计purport主旨，声称superior 长者, 高手, 上级，较高的, 上级的, 上好的, 出众的, 高傲的cumbersome 讨厌的, 麻烦的, 笨重的drastically 激烈地, 彻底地conservation 守衡律quadrature求积, 求积分interpolation插值extrapolation外推法, 推断internal point 内点generalized solution 广义解hydrodynamics 流体力学，水动力学divergence 发散（性），梯度，发散integro-interpolation method 积分插值法Variational method 变分方法comparatively 比较地, 相当地self-adjoint (nonself-adjoint) 自治的，自伴的，自共轭的finite element method 有限元法spline approximation 样条逼近Particles-in-the-Cell 网格质点法herald 使者, 传令官, 通报者, 先驱, 预兆，预报, 宣布, 传达, 欢呼advection水平对流fluctuation波动, 起伏mean-square 均方dispersion离差, 差量nterpolation 插值divisible可分的dice, die 骰子pitfall 缺陷celestial天上的macroscopic肉眼可见的, 巨观的classical field theory 经典场理论rigit 刚硬的, 刚性的, 严格的quantum量, 额, [物] 量子, 量子论inception 起初, 获得学位pertain 适合, 属于encompass 包围, 环绕, 包含或包括某事物ingredient 成分, 因素acquainted有知识的, 知晓的synonymous同义的configuration 构造, 结构, 配置, 外形inertia 惯性, 惯量attribute 特性momentum动量designate 指明projectile 射弹，发射的ballistics 弹道学, 发射学intractable 难处理的furnish 供应, 提供, 装备, 布置torque n. 扭矩, 转矩moment 力矩的dissipation 消散, 分散, 挥霍, 浪费, 消遣, 放荡, 狂饮constitutive构成的, 制定的continuum mechanics 连续介质力学superposition重叠, 重合, 叠合reckon 计算, 总计, 估计, 猜想，数, 计算, 估计, 依赖, 料想strength 强度load 载荷empirical 以经验为依据的insofar 在……范围cohesive 内聚性的stiffness 硬度furnish 供给turbulent 湍流laminar 层流isothermal 等温isotropic 各向同性eddy 旋涡viscosity 粘性、粘度adiabatic 绝热的reversible 可逆的isentropic 等熵的stream tube 流管tangential 切线的incompressible 不可压缩的similitude 相似性hydraulic 水力的,水力学的spillway (河或水坝的)放水道,泄洪道prototype 原型,样板vibratory 振动的,摆动的propagation 传播acoustic 听觉的,声学的damp 阻尼,衰减restore 复职,归还neutral 平衡exciting force 激励力resonant共振的,谐振的stiffness 刚度,刚性magnitude 数值,大小substantially实质上的perturb 干扰,扰乱Fourier series 傅里叶级数shredder 切菜器metropolitan 大都市的at-grade 在同一水平面上elevated 高架的guide way 导轨rigid body 刚体medium 介质aging 老化polymeric聚合(物)的consolidate 把…联合为一体,统一radically 根本地,本质上deliberate 从容不迫的,深思熟虑Attribute赋予medieval 中世纪的etch 蚀刻,蚀镂fingernail 指甲bar chart 直方图joystick 游戏杆trial-error 试制, 试生产junction n. 连接, 接合, 交叉点, 汇合处contrive v. 发明, 设计, 图谋snooker (=snooker pool)彩色台球, 桌球****公理axiom 命题proposition 被加数augend , summand 加数addend 被减数minuend 减数subtrahend 差remainder 被乘数multiplicand, faciend 乘数multiplicator 积product 被除数dividend 除数divisor 商quotient 大于等于is equal or greater than 小于等于is equal or lesser than 运算符operator 算术平均数geometric mean n个数之积的n次方根（reciprocal）x的倒数为1/x 有理数rational number 无理数irrational number 整数integer小数点decimal point分数fraction 分子numerator 分母denominator 比ratio 十进制decimal system 二进制binary system 十六进制hexadecimal system 权weight, significance 截尾truncation 四舍五入round 下舍入round down 上舍入round up 有效数字significant digit 无效数字insignificant digit 代数algebra 单项式monomial 多项式polynomial, multinomial 系数coefficient 未知数unknown, x-factor, y-factor, z-factor 等式，方程式equation 一次方程simple equation 二次方程quadratic equation 三次方程cubic equation 四次方程quartic equation 阶乘factorial 对数logarithm 指数，幂exponent 乘方power 二次方，平方square 三次方，立方cube 四次方the power of four, the fourth power n次方the power of n, the nth power 开方evolution, extraction 二次方根，平方根square root 三次方根，立方根cube root 四次方根the root of four, the fourth root n次方根the root of n, the nth root 坐标系coordinates 坐标轴x-axis, y-axis, z-axis 横坐标x-coordinate 纵坐标y-coordinate 原点origin 象限quadrant 截距(有正负之分)intercede （方程的）解solution 线段segment 射线radial 平行parallel 相交intersect 角度degree 弧度radian 钝角obtuse angle 平角straight angle 周角perigon 底base 锐角三角形acute triangle 直角边leg 斜边hypotenuse 勾股定理Pythagorean theorem 钝角三角形obtuse triangle 不等边三角形scalene triangle 等腰三角形isosceles triangle 等边三角形equilateral triangle 四边形quadrilateral 平行四边形parallelogram 周长perimeter 全等congruent 三角trigonometry 正弦sine 余弦cosine 正切tangent 余切cotangent 正割secant 余割cosecant 反正弦arc sine 反余弦arc cosine 反正切arc tangent 反余切arc cotangent 反正割arc secant 反余割arc cosecant 集合aggregate 空集void 子集subset 交集intersection 并集union 补集complement 映射mapping 定义域domain, field of definition 值域range 单调性monotonicity 图象image 数列，级数series 导数derivative 无穷小infinitesimal 复数complex number 矩阵matrix 行列式determinant 半圆semicircle 扇形sector 环ring 椭圆ellipse 圆周circumference 轨迹locus, loca(pl.) 平行六面体parallelepiped 立方体cube 七面体heptahedron 八面体octahedron 九面体enneahedron 十面体decahedron 十一面体hendecahedron 十二面体dodecahedron 二十面体icosahedron 多面体polyhedron 四面体tetrahedron 五面体pentahedron 六面体hexahedron 菱形rhomb, rhombus, rhombi(pl.), diamond 正方形square 梯形trapezoid 直角梯形right trapezoid 等腰梯形isosceles trapezoid 五边形pentagon 六边形hexagon 七边形heptagon 八边形octagon 九边形enneagon 十边形decagon 十一边形hendecagon 十二边形dodecagon 多边形polygon 正多边形equilateral polygon 相位phase 振幅amplitude 内心incentre(BrE), incenter(AmE) 外心excentre(BrE), excenter(AmE) 旁心escentre(BrE), escenter(AmE) 垂心orthocentre(BrE), orthocenter(AmE) 重心barycentre(BrE), barycenter(AmE) 内切圆inscribed circle 外切圆circumcircle 方差variance 标准差root-mean-square deviation, standard deviation 百分点percentage 百分位数percentile 排列permutation 分布distribution 正态分布normal distribution 非正态分布abnormal distribution 条形统计图bar graph 柱形统计图histogram 折线统计图broken line graph 曲线统计图curve diagram 扇形统计图pie diagram**** mutually disjoint events 互不相交事件mutually disjoint subsets 互不相交子集mutually independent events 互相独立事件myria 万myriad 无数的multiplicity 重数mid square method 平方取中法midperpendicular 中垂线minor 子式minor arc 劣弧mixed number 带分数regular convergence 正则收敛relative discriminant 相对判别式relative error 相对误差relative extremum 局部极值ricci equatoin 李奇恒等式ricci identity 李奇恒等式riemann function 黎曼函数riemann integral 黎曼积分right direct product 右直积right endpoint 右端点right inner product 右内积ring of integers 整数环ring of matrices 矩阵环root mean square error 均方根差root of equation 方程式的根rotation of axes 坐标轴的旋转rotation of co ordinate system 坐标轴的旋转round off error 舍入规则round up error 舍入规则runge kutta method 龙格库塔法n disk n维圆盘nth member 第n项nth partial quotient 第n偏商nth power operation n次幂运算nth root n次根nth term 第n项n times continuously differentiable n次连续可微的natural injection 自然单射natural isomorphism 自然等necessary and sufficient conditions 必要充分的条件necessary and sufficient statistic 必要充分统计量neutral element 零元素neutral line 中线nonhomogeneous linear boundary value problem 非齐次线性边值问题nonhomogeneous linear differential equation 非齐次线性微分方程nonhomogeneous linear system of differential equations 非齐次线性微分方程组interval algebra 区间代数interval analysis 区间分析interval closed at the right 右闭区间interval estimation 区域估计interval function 区间函数interval graph 区间图interval of convergence 收敛区间interval of definition 定义区间interval topology 区间拓扑irreducible set 不可约集irreducible r module 不可约r模periodical decimal fraction 循环十进小数pentad 拼五小组pentadecagon 十五边形pentagon 五角形pentagonal number 五角数pentagonal pyramid 五角锥pentagram 五角星pentahedron 五面体pentaspherical coordinates 五球坐标penalty method 补偿法pascal distribution 帕斯卡分布partition function 分折函数partial differential equation of elliptic type 椭圆型偏微分方程partial differential equation of first order 一阶偏微分方程partial differential equation of hyperbolic type 双曲型偏微分方程partial differential equation of mixed type 混合型偏微分方程partial differential equation of parabolic type 抛物型偏微分方程partial differential operator 偏微分算子parametric test 参数检验particular solution 特解parallelogram axiom 平行四边形公理orthogonality relation 正交关系ordinary differential equation 常微分方程optimal value function 最优值函数opposite angles 对角opposite category 对偶范畴one to one mapping 一一映射onto mapping 满射open mapping theorem 开映射定理one to many mapping 一对多映射one sided limit 单侧极限numerical solution of linear equations 线性方程组的数值解法null set 空集null solution 零解third boundary condition 第三边界条件two sided neighborhood 双侧邻域unbiased estimating equation 无偏估计方程unbounded function 无界函数unbounded quantifier 无界量词uncertainty principle 测不准原理uncorrelated random variables 不相关随机变量undetermined coefficient 末定系数velocity distribution 速度分布velocity optimal 速度最优的weak approximation theorem 弱逼近定理weak completeness 弱完备性weak continuity 弱连续性weak convergence 弱收敛wiener measure 维纳测度word group 自由群sample correlation coefficient 样本相关系数sample covariance 样本协方差schwarz inequality 施瓦尔兹不等式second boundary condition 诺伊曼边界条件second comparison test 第二比较检验second limit theorem 第二极限定理self adjoint differential equation 自伴微分方程semimajor axis 半长轴semiminor axis 半短轴sentential calculus 命题演算set of measure zero 零测度集set topology 集论拓扑simple connectedness 单连通性slope function 斜率函数solution curve 积分曲线solution domain 解域solution set of equation 方程的解集spatial co ordinate 空间坐标specific address 绝对地址spherical bessel function 球贝塞耳函数spherical cap 球冠spherical coordinates 球极坐标spherical curvature 球面曲率spherical shell 球壳spherical zone 球带spline function 样条函数spline interpolation 样条内插stability conditions 稳定条件statistical hypothesis testing 统计假设检验strict inequality 严格不等式strict isotonicity 严格保序性strict isotony 严格保序性strict increasing 严格递增system of partial differential equations 偏微分方程组system of ordinary differential equations 常微分方程组system of linear homogeneous equations 线性齐次方程组system of linear inhomogeneous equations 线性非齐次方程组system of inequalities 联立不等式system of polar coordinates 极坐标系system of variational equations 变分方程组system with concentrated parameters 集中参数系统system with distributed parameters 分布参数系统t1topological space t1拓扑空间t2topological space t2拓扑空间t3topological space 分离空间t4topological space 正则拓扑空间t5 topological space 正规空间t6topological space 遗传正规空间tangent cone 切线锥面telegraph equation 电报方程theorem for damping 阻尼定理****充分条件sufficient condition必要条件necessary condition 充要条件sufficient and necessary condition……的充要条件是………if and only if …****abscissa 横坐标alternating series 交错级数angle of the sector 扇形角arbitrary constant 任意常数augmented matrix 增广矩阵axis of parabola 拋物线的轴axis of revolution 旋转轴axis of rotation 旋转轴binomial series 二项级数binomial theorem 二项式定理binomial distribution 二项分布bisection method 分半法；分半方法bounded above 有上界的；上有界的bounded below 有下界的；下有界的bounded function 有界函数bounded sequence 有界序列brace 大括号bracket 括号Cartesian coordinates 笛卡儿坐标certain event 必然事件circumcentre 外心；外接圆心circumcircle 外接圆classical theory of probability 古典概率论cofactor 余因子; 余因式common denominator 同分母；公分母common difference 公差common divisor 公约数；公约common logarithm 常用对数common multiple 公位数；公倍common ratio 公比commutative law 交换律compasses 圆规Cauchy-Schwarz inequality 柯西- 许瓦尔兹不等式central limit theorem 中心极限定理centripedal acceleration 向心加速度concave downward 凹向下的concurrent 共点concyclic 共圆concyclic points 共圆点Euclidean geometry 欧几里德几何Euler's formula 尤拉公式；欧拉公式even function 偶函数even number 偶数game （1）对策；（2）博奕Gaussian distribution 高斯分布greatest term 最大项greatest value 最大值harmonic mean (1) 调和平均数; (2) 调和中项harmonic progression 调和级数higher order derivative 高阶导数improper fraction 假分数improper integral 广义积分; 非正常积分implicit function 隐函数incircle 内切圆inclined plane 斜included angle 夹角indefinite integral 不定积分initial condition 原始条件；初值条件initial-value problem 初值问题interior angles on the same side of the transversal 同旁内角interior opposite angle 内对角isosceles triangle 等腰三角形iterate (1)迭代值; (2)迭代Lagrange interpolating polynomial 拉格朗日插值多项代Laplace expansion 拉普拉斯展式lemniscate 双纽线left hand limit 左方极限limiting case 极限情况limiting position 极限位置line of best-fit 最佳拟合line segment 线段logarithmic equation 对数方程mathematical analysis 数学分析mathematical induction 数学归纳法monotonic decreasing function 单调递减函数monotonic convergence 单调收敛性monotonic increasing function 单调递增函数multiple-angle formula 倍角公式multiple root 多重根mutually disjoint 互不相交mutually exclusive events 互斥事件mutually independent 独立; 互相独立mutually perpendicular lines 互相垂直numerical method 计算方法；数值法oblique cone 斜圆锥orthogonal circles 正交圆orthogonality 正交性oscillatory convergence 振动收敛性ordinary differential equation 常微分方程pairwise mutually exclusive events 两两互斥事件place holder 补位数字point of inflection (inflexion) 拐点; 转折点Pisson distribution 泊松分布point-slope form 点斜式polar coordinate plane 极坐标平面polynomial equation 多项式方程posterior probability 后验概率; 事后概率premultiply 前乘; 自左乘prime factor 质因子；质因素prime number 素数；质数principal angle 主角principal axis 主轴principal value 主值prior probability 先验概率; 事先概率probability density function 概率密度函数product and sum formula 和积互变公式product sample space 积样本空间product to sum formula 积化和差公式proof by contradiction 反证法; 归谬法proper fraction 真分数proper integral 正常积分proper subset 真子集propositional calculus 命题演算propositional inference 命题推演protractor 量角器Pythagoras' theorem 勾股定理Pythagorean triplet 毕氏三元数组quadratic convergence 二阶收敛性quadrature 求积法quotient set 商集radial component 沿径分量radical axis 根轴range 值域；区域；范围；极差；分布域rationalization 有理化raw data 原始数据rectifiable 可求长的reciprocal 倒数rectangular coordinate plane 直角坐标平面recurrence formula 递推公式reducibility 可约性; 可化简性reflexive relation 自反关系reference angle 参考reference line 基准线reflex angle 优角；反角region of acceptance 接受区域region of convergency 收敛区域region of rejection 否定区域right circular cone 直立圆锥（体）resolution of vector 向量分解; 矢量分解right hand limit 右方极限right prism 直立棱柱；直立角柱(体) right pyramid 直立棱锥；直立角锥(体) right-angled triangle 直角二角形scalene triangle 不等边三角形；不规则三角形scatter diagram 散点图scientific notation 科学记数法semi-conjugate axis 半共轭轴semi-transverse axis 半贯轴semi-vertical angle 半顶角separable differential equation 可分微分方程septic equation 七次方程set square 三角尺；三角板shaded portion 有阴影部分significance level 显著性水平significant figure 有效数字similar triangles 相似三角形simple iteration method 简单迭代法simple pendulum 单摆Simpson's integral 森逊积分standard deviation 标准差；标准偏离standard normal distribution 标准正态分布; 标准常态分布stationary point 平稳点; 逗留点; 驻点strictly monotonic 严格单调statistical chart 统计分析submultiple angle formula 半角公式subsidiary angle 辅助角substitution 代入; 代入法successive approximation 逐次逼近法successive derivative 逐次导数successive differentiation 逐次微分法suffix 下标sum to infinity 无限项之和sum to product formula 和化积公式superimposing 迭合supplementary angle 补角surjection 满射symmetric relation 对称关系tautology 恒真命题；恒真式Taylor’s expansion 泰勒展开式Taylor’s series 泰勒级数Taylor’s theorem 泰勒定理test criterion 检验标准test of significance 显著性检验to the nearest 至最接近之torque 转矩torus 环面transcendental function 超越函数transformation of variable 变数转换transitive 可传递的transpose of matrix 倒置矩阵；转置矩阵transversal 截；横截的triangle law of addition 三角形加法travel graph 行程图tree diagram 树形图trapezoidal integral 梯形积分truncated Taylor’s series 截断泰勒级数two-tailed test 双尾检验；只端检验type I error I型误差type II error II型误差unbiased estimator 无偏估计量undetermined coefficient 待定系数unique solution 唯一解vertical asymptote 垂直渐近线vertically opposite angles 对顶角without loss of generality 不失一般性****分子Numerator 分母Denominator 阿拉伯数字Hindu-Arabic numeral假分数Improper fraction 最大公因子Highest Common Factor (H.C.F.) 最小公倍数Lowest Common Multiple (L.C.M.) 行列式determinant****interval closed at the right 右闭区间interval of convergence 收敛区间interval of definition 定义区间invariance theorem 不变性定理invariant of an equation 方程的不变量inverse circular function 反三角函数inverse hyperbolic function 反双曲函数inversion formula 反演公式isotonic injective mapping 保序单射映射jacobi identity 雅可比恒等式jump point 跳跃点law of double negation 双重否定律law of inertia 惯性律law of large numbers 大数定律leading ideal 猪想liouville theorem 刘维尔定理lipschitz condition 李普希茨条件markov transform 马尔可夫变换mathematical approximation 数学近似法mathematical model 数学模型maximum condition 极大条件maximum deviation 最大偏差mean square deviation 方差mean square of error 误差的均方meromorphic function 亚纯函数柱形统计图histogram 折线统计图broken line graph 曲线统计图curve diagram 扇形统计图pie diagram排列permutatio内切圆inscribed circle 外切圆circumcircle正多边形equilateral polygon metric space 度量空间metric subspace 度量子空间method of runge kutta type 朗格库塔型的方法method of steepest ascent 最速上升法method of steepest descent 最速下降法method of finite elements 有限元法method of fractional steps 分步法method of exhaustion 穷竭法method of approximation 近似法method of artificial variables 人工变量法method of balayage 扫除法method of characteristic curves 特者法method of comparison 比较法method of conjugate gradients 共轭梯度法lateral area 侧面积last multiplier 最后乘子large sample test 大样本检验lattice constant 点阵常数lattice design 格子设计method of difference 差分法method of elimination 消元法method of estimation 估计法meromorphic differential 亚纯微分median 中位数measuring rule 量尺mean term 内项mean term 内项mean term 内项irreducibility criterion 不可约性判别准则irreducible polynomial 不可约多项式irreducible generating set 不可约生成集irregular divisor class 非正则因子类irregular point 非正则点irregular singular point 非正则奇点isometric circle 等距圆isometric embedding 等距嵌入isomorphic field 同构域isomorphic graph 同构图isomorphic group 同构群isomorphic image 同构象isothermal parameter 等温参数iterated function 叠函数iterated integral 累积分joint distribution 联合分布jordan algebra 约当代数kernel of an integral equation 积分方程的核l'hospital rule 洛必达规则laboratory system of coordinates 实验室坐标系labyrinth 迷宫lacation principle 介值定理lag correlation coefficient 滞后相关系数lag regression 落后回归laguerre differential equation 拉盖尔微分方程lame equation 拉梅方程language of formula 公式语言laplace beltrami operator 拉普拉斯贝尔特拉米算子lateral area 侧面积last multiplier 最后乘子large sample test 大样本检验lattice constant 点阵常数lattice design 格子设计left adjoint 左伴随的left derivative 左导数left differential 左微分left direct product 左直积left end point 左端点left length 左长left limit value 左极限值left multiplication ring 左乘环length of curve 曲线的长length of normal 法线的长levi decomposition 列维分解limes inferior 下极限limes superior 上极限limit circle 极限圆limit circle type 极限圆型logarithm to the base 10 常用对数logarithmic normal distribution 对数正态分布logic of relations 关系逻辑magic circle 幻圆magic cube 幻立方manifold without boundary 无边廖many valued mapping 多值映射marginal distribution density function 边缘分布密度函数marginal distribution function 边缘分布函数mathematical programming 数学规划mathematical random sample 数学随机样本mathematical statistics 数理统计maximum likelihood estimating function 极大似然估计量independent variable 自变量dependent variable 应变量equiangular triangle 正三角形,等角三角形命题proposition 差remainder 积product 除数divisor 商quotient 截尾truncation 未知数unknown, x-factor, y-factor, z-factor 阶乘factorial 集合aggregate 空集void 子集subset 交集intersection 并集union 补集complement 映射mapping 勾股定理Pythagorean theorem 菱形rhomb, rhombus, rhombi(pl.), diamond 双曲线hyperbola 抛物线parabola topology of bounded convergence 有界收敛拓扑toroid 超环面toroidal coordinates 圆环坐标trace of dyadic 并向量的迹transcendental integral function 超越整函数transformation formulas of the coordinates 坐标的变换公式transformation to principal axes 轴变换transversal lines 截线trapezoid method 梯形公式trefoil knot 三叶形纽结truth function 真值函项two sided test 双侧检定two sided neighborhood 双侧邻域two sided surface 双侧曲面two termed expression 二项式ultrahyperbolic equation 超双曲型方程a priori bound 先验界限a priori distribution 先验分布a priori probability 先验概率a summable a可和的abacus 算盘abbreviate 略abbreviation 简化abel equation 阿贝耳方程abel identity 阿贝耳恒等式abel inequality 阿贝耳不等式abel summation method 阿贝耳求和法abelian algebra 阿贝耳代数abelian differential 阿贝耳微分abelian equation 阿贝耳方程abelian extension 阿贝耳扩张abelian function 阿贝耳函数abelian function field 阿贝耳函数域abelian functor 阿贝耳函子abelian group 交换群abelian groupoid 阿贝耳广群abelian integral 阿贝耳积分abelian summation 阿贝耳求和法abelian theorem 阿贝耳定理abelian variety 阿贝耳簇abridge 略abridged notation 简算记号abscissa 横坐标abscissa of absolute convergence 绝对收敛坐标abscissa of summability 可和性坐标abscissa of uniform convergence 一致收敛横坐标absolute 绝对形absolute address 绝对地址absolute class field 绝对类域absolute coding 绝对编码absolute cohomology 绝对上同调absolute conic 绝对二次曲线absolute convergence 绝对收敛absolute curvature vector 绝对曲率向量absolute deviation 绝对偏差absolute differential calculus 绝对微分学absolute error 绝对误差absolute extremes 绝对极值absolute extremum 绝对极值absolute frequency 绝对频率。

数学英语词汇(初等数学)

初等数学——Elementary mathematics基本数学名词公式 Formula推论 Corollary；Deduction已知 Known准则 Criterion定义 Definition公设 Postulate引理 Lemma命题 Propositiontheorem 基本定理 Fundamentaltheorem逆定理 Inverseproposition；Converse proposition 逆命题 Inverse等价 Equivalencerelation；Relation of equivalence 等价关系 Equivalentcondition 充分条件 Sufficientcondition 必要条件 Necessary充分必要条件Necessary and sufficient condition悖论 Paradox基本数学方法induction 数学归纳法 Mathematical递推公式 Recurrenceequation 反证法Proof of contradiction归纳证明Proof of induction几何方法 Methodgeometryofparabolas 抛物线法 Methodof置换法Method of substitution加权平均法Method of weighted mean数整数 Integer正的 Positiveinteger正整数 Positivenumber 正数 Positive负的 Negative负号 Negativesignnumber 负数 Negativenumber偶数 Evennumber奇数 Odd奇偶 Odd-evendigit奇偶数字 Paritynumber有理数 Rational无理数 Irrationalnumber 实的 Real实数 Realnumberaxis实轴 Realnumber虚数 Imagineaxis虚轴 Imaginary纯虚数Purely imaginary numberunit虚数单位 Imaginarynumber复数 Complex共轭复数Conjugate complex number模 Moduli；Modulo；Modulus复数的模Modulus of complex number域 Field；Domain有理数域Field of rational numbersnumber素数 Prime互素 Coprime绝对值 Absolutevalue十进制 Decade分数 Fraction；Fractional numberstroke分数线 Fractionfraction真分数 Properfraction带分数 Mixedfraction简分数 Simple百分数 Percentage比率 Ratiodecimal循环小数 Periodicpoint小数点 Radix有效数字 Significantfigure倒数Inverse of a number；Reciprocal分子 Numerator分母 Denominatordenominator公分母 Commondivisor公约数 Common公倍数 Commonmultiplemultiple；Lowest common multiple 最小公倍数 Leastcommon代数——Algebra四则运算Four arithmetic operationrule运算法则 Operational加法 Addition减、负 Minus商 Quatient因子 Factor；Divisor因式分解 Factoringfactor公因子 Common最大 Maximum；Maximalvalue；Greatest value最大值 Maximal最大点 Maximumpoint最小 Minimum；Minimalvalue最小值 Minimal最小点 Minimalpointfactorcommon最大公因子 Greatestnumber未知数 Unknown方程 Equationn次方程Equation of n-th order方程组Set of equations；System of equationsequations联立方程 Simultaneous不等式 Inequality判别式 Discriminant根 Rootroot单根 Simpleroot相异根 Distinctroot二重根 Doublenumbers重数 Multipleroot；Repeated root重根 Multiple根的重数 Multiplicityrootofroot虚根 Imaginary映射 Mapmapping逆映射 Inversemapping一一映射 One-to-one一一对应 Monogamy；One-to-one correspondence函数 Functionfunction；One-valued function；Monotropic function 单值函数 Monodromefunction；Many-value function；Multi-valued function 多值函数 Multiformvariable自变量 Independentmethod图示法 Graphical一元函数Function of a single variable一次函数Function of first degreevariablesmany多元函数 Functionofn-variablesn元函数 Functionofterm高次项 Higher隐式 Implicitfunction隐函数 Implicitfunction显函数 Explicit定义域Domain of definition；Field of definitionfunction函数的值域 Rangeof复合 Composition复合函数 Compositefunctionfunction反函数 Inverse奇偶性 Odevity；Parity偶函数 Evenfunctionfunction奇函数 Odd周期 Period常数 Constant变量 Variable；Variant参数 Parameterequation参数方程 Parameter参变量 Parametricvariablefunction线性函数 Linear幂 Power幂数Power of a numberfunction幂函数 Power二次的 Quadratic平方 Squaresum平方和 Quadratic开平方Extraction of square root；Rootingroot；Quadratic root平方根 Square正平方根Positive square root根号 Radical被开方数 Radicand开方 Radication立方 Cubic；Third power开立方Extraction of cubic rootroot立方根 Cubic阶乘 Factorial系数 Coefficientpower 乘方 Mathematical次数 Degree二次函数 Quadraticfunctionexpression 整式 Integralterm二次项 Quadraticexpression 单项式 Monomial多项式 Polynomial；Multinomialn次多项式Polynomial of degree npolynomialof多项式的次数 Degree多项式的项Term of a polynomialelement首项元素 Leading多项式相除法Division algorithm for polynomial有理分式 Rationalfraction指数 Exponentfunction指数函数 Exponentialcurve；Exponent curve指数曲线 Exponential对数 Logarithmfunction对数函数 Logarithmbase对数的底 Logarithmiclogarithm常用对数 Commonlogarithm；Napierian logarithms自然对数 Naturallogarithm 反对数 Inversefunction三角函数 Triangle正弦 Sinecurve正弦曲线 Sinusoidal余弦 Cosine正切 Tangent余切 Cotangent正割 Secant余割 Cosecantvalue 主值 Principalfunctioncircular反三角函数 Inversesine；Inverse sine反正弦 Arccosine反余弦 Arctangent反正切 Arccotangent反余切 Arcsecant反正割 Arccosecant反余割 Arc弧度 Radian幅角 Argumentlaw结合律 Associativelaw 消去律 Cancellation交换律 Commutativelawlaw分配律 Distributivelaw对偶律 Dualizationaverage；Geometric mean几何平均 Geometric平均值 Mean；Mean value；Averagenumber平均数 Meanvelocity平均速度 Meanelement单位元素 Identicalmean算术中项 Arithmeticcut黄金分割 Golden集合 Set元素 Element集合的元素Element of set空集 Emptyset集合的包含Inclusion of sets子集 Subset；Subaggregate真子集 Propersubsetinclude真包含 Proper并 Union集合的并Union of sets；Set unionaggregate 并集 Sum交 Intersectionsets；Product of sets集合的交 Intersectionofset无穷集 Infiniteset；Supplementary补集 Complementaryset开集 Openset闭集 Closed区间 Intervalinterval开区间 Open闭区间 Closedinterval有限的 Finiteinterval有限区间 Finite有限开区间Finite open interval无限的 Infinite；Boundlessinterval无穷区间 Infinite端点 EndpointTheorem二项式定理 Binomialdifference 公差 Common等比 Equalratio；Ratio of equalityratio公比 Common排列数Number of permutations排列 Permutation组合 Combinationoperation逆运算 Inverse无顺序 Out-of-order几何——Geometrygraph平面图形 Planar平面 Planegeometry平面几何 Planespace 二维空间 Two-dimensional三维空间 Third-dimensionalspaceline直线 Straight铅垂线 Plumb-line斜率 Gradient；Slope截距 Intercept交点 Intersectionpointoftangency 切点 Pointline；Tangential line切线 Tangent曲线的切线方程Tangential equation of a curve点斜式Point slope form直线 Line垂线 Perpendicular垂直 Perpendicularityline法线 Normal平行 Parallelstraightlines平行直线 Parallelline有向直线 Directedsegment线段 Line线段长度Length of segmentlength单位长度 Unit射线 Ray角 Angleangle锐角 Acuteangle 倾角 Inclinationangle 夹角 Included旋转 Pivoting圆 Circlecircle单位圆 Unit圆心Center of a circle圆周 Circumferencering圆环 Circular圆心角Angle at the centre圆周角Angle in a circular segmentcylinder 圆柱 Circularcone圆锥 Circular周长 Perimeter直径 Diameter半径 Radius内径 Inradiusdiameter；Outside diameter外径 Outercircle内切圆 Inscribed弧长Length of arc半圆 Semi-circle扇形 Sector三角形 Triangle等腰三角形 Isoscelestriangle等腰直角三角形Isosceles right triangletriangle等边三角形 Equilateraltriangle直角三角形 Righttriangle锐角三角形 Acuteangle钝角 Obtusetriangle钝角三角形 Obtuse相似三角形 Similartrianglespoint；Middle point；Mid-point中点、重心 Median垂心 Orthocenter矩形 Rectangletrapezoid等腰梯形 Isosceles平行四边形 Parallelogram菱形 Lozenge；Diamond；Rhomb多边形 Polygonline对角线 Diagonal辅助线 Auxiliaryline顺时针方向 Clockwise反时针方向 Anti-clockwisespace欧几里德空间 Euclideanline水平线 Horizontal水平面 Horizontalplane坐标 Coordinate原点 Origin坐标轴 Coordinateaxisplanes坐标平面 Coordinatesystem坐标系 Coordinate直角 Rightanglecoordinates 直角坐标 Rectangular直角坐标系Rectangular coordinate system轴 Axis横坐标 Abscissaaxis横轴 Abscissa纵轴 Ordinatesaxis；Longitudinal axisaxis竖轴 Vertical半轴 Semi-axispolarcoordinates；Polar coordinates极坐标 Planeaxis极轴 Polarradius极半径 Polar极点 Pole极坐标的极点Pole of polar coordinates维 Dimensionline曲线 Curve对称 Symmetric对称轴Axis of symmetry不对称的 Unsymmetricaltransformation 坐标变换 Coordinatesurface曲面 Curve共面 Coplane立方体 Cubecurve；Quadric curve二次曲线 Quadratic抛物线 Parabola椭圆 Ellipseaxis；Prolate axis长轴 Major双曲线 Hyperbola退化双曲线 Degeneratehyperbola焦点 Focus渐近线 Asymptote；Asymptotic line空间几何Geometry of spacesurface横截面 Transversal球形 Sphere球冠 Sphericalcapcrown球冠 Spherical球的大圆Great circle of a sphere柱 Cylindersurface 柱面 Cylindricalsurface；Quadric surface二次曲面 Quadraticcylinder二次柱面 Quadric。

IQview使用说明

Menu Items Save PSDU data
Description
Saves the captured data of the packet to a text file. Two options exist: • Packet Info PSDU: Saves the data of the complete packet. • PSDU: Saves the data of the PSDU (data) portion of the packet only
Main Menu－File Menu
Menu Items Load Default Setup Exit
Descriptionngs for the application. Exits the program.
Software operation-IQ Signal
Software operation-IQ Signal
Main Menu－Setup Menu-
parameters
OFDM Optimization Parameters
Description
192.168.100.X, 其中X是1到253之間的任意一個整數 (IQview的該位址是254) 5. 在子网掩碼一欄中輸入255.255.0.0 點繫確定 , 便完成設置
IQview安裝與設定
安裝IQsignal 802.11 Tester 測試軟件
插入 IQview Applications CD-ROM 光盤, 窗口會出現安裝畫面
Software operation-IQ Signal
IQ Signal主界面-功能畫面
Top-Right測量界面
Software operation-IQ Signal

某咨询国际的中英文双语作品国际职位评估系统

Similar activities within a function/unit 在同一功能组别内,担任相似的工作
Diverse activities within different functions/units 在不同功能组别内,担任不同的工作
Position holder decides what-, why-,when-,where- and how to do for the organization 任职人为机构制定作什么,为何作, 何时作,那里作和如何作
Position holder sets overall targets and policies for the corporation in consultation with the Corporate Board 谘询集团董事会后, 任职人为集团制定全面的目标和政策
解决问题难度Problem solving
环境条件Environmental conditions
JOB COMPLEXITY 工作复杂程度
职位评估系统七个因素的比重
The Weighting of IPE Factors
解决问题难度
任职资格
沟通技巧
环镜条件
对企业的影响
监督管理
责任范围
总分数Total Points:65-1193
Headcount of subordinates : 43下属人数 : 43
下属人数 (直接和间接的)
Number of Subordinates (direct and indirect)
gives an indication of the management qualifications required 明确管理者所具备的资格consider the total number of employees for which the postion is responsible, both directly reporting and those reporting through subordinates 包括所有监督的职员,直接报告的和通过下属间接报告的人也计算在内when subordinates clearly have two reporting lines, then the number of such subordinates should be divided by two 当下属清楚地向两方上级报告, 将这类下属的人数除二

图论总结(超强大)解读

1.图论Graph Theory1.1.定义与术语Definition and Glossary1.1.1.图与网络Graph and Network1.1.2.图的术语Glossary of Graph1.1.3.路径与回路Path and Cycle1.1.4.连通性Connectivity1.1.5.图论中特殊的集合Sets in graph1.1.6.匹配Matching1.1.7.树Tree1.1.8.组合优化Combinatorial optimization1.2.图的表示Expressions of graph1.2.1.邻接矩阵Adjacency matrix1.2.2.关联矩阵Incidence matrix1.2.3.邻接表Adjacency list1.2.4.弧表Arc list1.2.5.星形表示Star1.3.图的遍历Traveling in graph1.3.1.深度优先搜索Depth first search (DFS)1.3.1.1.概念1.3.1.2.求无向连通图中的桥Finding bridges in undirected graph1.3.2.广度优先搜索Breadth first search (BFS)1.4.拓扑排序Topological sort1.5.路径与回路Paths and circuits1.5.1.欧拉路径或回路Eulerian path1.5.1.1.无向图1.5.1.2.有向图1.5.1.3.混合图1.5.1.4.无权图Unweighted1.5.1.5.有权图Weighed —中国邮路问题The Chinese post problem1.5.2.Hamiltonian Cycle 哈氏路径与回路1.5.2.1.无权图Unweighted1.5.2.2.有权图Weighed —旅行商问题The travelling salesman problem1.6.网络优化Network optimization1.6.1.最小生成树Minimum spanning trees1.6.1.1.基本算法Basic algorithms1.6.1.1.1.Prim1.6.1.1.2.Kruskal1.6.1.1.3.Sollin（Boruvka）1.6.1.2.扩展模型Extended models1.6.1.2.1.度限制生成树Minimum degree-bounded spanning trees1.6.1.2.2.k小生成树The k minimum spanning tree problem(k-MST)1.6.2.最短路Shortest paths1.6.2.1.单源最短路Single-source shortest paths1.6.2.1.1.基本算法Basic algorithms1.6.2.1.1.1. ..................................................................................................... D ijkstra1.6.2.1.1.2. .......................................................................................... B ellman-Ford1.6.2.1.1.2.1.....................................Shortest path faster algorithm(SPFA)1.6.2.1.2.应用Applications1.6.2.1.2.1. ........................... 差分约束系统System of difference constraints1.6.2.1.2.2. .......................... 有向无环图上的最短路Shortest paths in DAG1.6.2.2.所有顶点对间最短路All-pairs shortest paths1.6.2.2.1.基本算法Basic algorithms1.6.2.2.1.1. ....................................................................................... F loyd-Warshall1.6.2.2.1.2. .................................................................................................... Johnson 1.6.3.网络流Flow network1.6.3.1.最大流Maximum flow1.6.3.1.1.基本算法Basic algorithms1.6.3.1.1.1. ........................................................................ Ford-Fulkerson method1.6.3.1.1.1.1.......................................................... E dmonds-Karp algorithm1.6.3.1.1.1.1.1. ................................................... M inimum length path1.6.3.1.1.1.1.2. ........................................... Maximum capability path1.6.3.1.1.2. ............................................... 预流推进算法Preflow push method1.6.3.1.1.2.1.................................................................................. P ush-relabel1.6.3.1.1.2.2........................................................................... Relabel-to-front1.6.3.1.1.3. .......................................................................................... Dinic method1.6.3.1.2.扩展模型Extended models1.6.3.1.2.1. ............................................................................... 有上下界的流问题1.6.3.2.最小费用流Minimum cost flow1.6.3.2.1.找最小费用路Finding minimum cost path1.6.3.2.2.找负权圈Finding negative circle1.6.3.2.3.网络单纯形Network simplex algorithm1.6.4.匹配Matching1.6.4.1.二分图Bipartite Graph1.6.4.1.1.无权图-匈牙利算法Unweighted - Hopcroft and Karp algorithm1.6.4.1.2.带权图-KM算法Weighted –Kuhn-Munkres(KM) algorithm1.6.4.2.一般图General Graph1.6.4.2.1.无权图-带花树算法Unweighted - Blossom (Edmonds)1.图论Graph Theory1.1. 定义与术语Definition and Glossary1.1.1.图与网络Graph and Network二元组(),V E称为图(graph)。

CCA

sets that is optimal in a correlation sense. It has been shown that ﬁnding the canonical correlations is equivalent to maximizing the mutual information between the sets if the underlying distributions are elliptically symmetric [9]. Consider two random variables, x and y, from a multi-normal distribution. ConT sider the linear combinations, x = wT x (x − x) and y = wy (y − y), of the two variables respectively. x denotes the mean of x. The correlation between x and y is given by ρ= wT x Cxy wy
3
Learning visual operators from examples
The basic idea behind the proposed method, illustrated in ﬁgure 1, is to analyse two signals where the feature that is to be represented generates dependent signal components. The signal vectors fed into the CCA are image data mapped through a function f . In general, f can be any vector-valued function of the image data. The choice of f is of major importance as it determines the representation of input data for the canonical correlation analysis. It is f that gives the desired invariance properties. Other authors have proposed nonlinear extensions to CCA, which includes f in the learning process [1, 10]. In this case, however, we have used a ﬁxed function f . The training data are presented in pairs such that the features for which we want to ﬁnd a representation vary in a correlated way. Other features, for which we want the representation to be invariant to, are varied in an unordered way. In this way, the desired features are captured by the CCA.

Datalogic 激光扫描仪和光幕用户手册说明书

Overview
Safety devices are essential for a safe work environment. They can be used in various configurations to monitor safety zones where access is limited. Only one safety device (laser scanner or light curtains) can be connected to Vention’s Safety Module at a time. If you need to interface N number of safety devices to MachineMotion V2, you will need N number of safety modules (daisy-chained). Please refer to the User Manual for more information about the connections.
To set parameters, select Configuration to access a drop-down menu. For each item on the drop-down menu, enter the desired values in the appropriate fields (highlighted in yellow). More details are provided for each parameter below.
Maximum safe zone: 5.5 m radius. Maximum warning zone: 40 m radius. Detection angle: 275o. Total/partial dynamic muting available. Zone switching (bank switching) available.

XOR-based

The placement ofmatrices when usingXOR-based hashingBavo Nootaert∗,Hans Vandierendonck∗,Koen De Bosschere∗∗ELIS,Ghent University,Sint-Pietersnieuwstraat41,9000Gent,BelgiumABSTRACTTo reduce the number of conﬂicts in caches,hash function are used.One family of hash function that has been reported to perform well in the presence of stride patterns are based on XOR-ing address bits together.We show that,in contrast to traditional modulo-2m-based hashing,for XOR-based hashing the placement of stride patterns and matrices has a signiﬁcant impact on conﬂicts. KEYWORDS:cache,optimization,stride pattern,XOR-based hash function1IntroductionHash functions have been studied with the aim of avoiding conﬂict misses in caches[Schl93, Gonz97,Toph99].In scientiﬁc programs,stride patterns are common.It is important to choose a hash function so as to map as many strides as possible with few conﬂicts.Conven-tional bit selection has the disadvantage of mapping even strides onto only one halve of the sets.A well studied alternative are the XOR-based hash functions[Frai85,Gonz97,Vand05], and a special subclass thereof,the polynomial hash functions[Rau91].These functions are computed using arithmetic over GF(2)and can be evaluated using solely XOR-gates.In[Toph99]benchmarks are used to measure the performance of these hash functions. Analytical studies of XOR-based hash functions are usually limited to vector space pat-terns[Frai85,Vand05].Analyzing these patterns is rather restrictive,since this class con-tains only certain strides,aligned to certain base addresses.In[Rau91],some properties are proven about the effect of polynomial hash functions on stride patterns starting at base ad-dress0.Although some of the theorems can be extended to include a different base address [Noot05],they are aimed at determining equivalence among patterns,and generally can-not say whether a particular base address is actually a good choice.Indeed,the number of conﬂicts varies greatly depending on the alignment of the stride pattern.In Section2we model self interference in a matrix using stride patterns.In Section3 we use this model to show the potential impact of the base address,independent of any particular benchmark.10000 1500020000 25000 300000 2e+06 4e+06 6e+068e+06 1e+07n u m b e r o f c o n f l i c t s base address Figure 1:The number of conﬂicts for a 976×976matrix.2A model for self interference in a matrixWe focus on self interference [Lam91]in a matrix:if the cache is large enough,cross inter-ference can be ignored.Assume interference is mainly restricted to within separate columns or rows of a matrix.This assumption is valid if each column or row is reused repeatedly,without intermediate accesses to other columns of the same matrix.A matrix can now be considered as a collection of stride patterns.An S ×S matrix stored in row major order is made of S patterns with stride S (the columns),and S with stride 1(the rows).Let a be the starting address of a matrix A .The patterns with stride S start at addresses a ,a +1,...,a +S −1,and the others start at addresses a ,a +S ,...,a +(S −1)S .A conﬂict occurs when an element of a pattern is mapped to a set that already contains an element.The number of conﬂicts is independent of the order in which the elements are mapped.We deﬁne the number of conﬂicts of a matrix as the sum of the conﬂicts of the column access patterns and the row access patterns.3An exampleConsider a cache with 213sets and a mapping that XORs two slices of 13bits together,and discards any bits above the 26least signiﬁcant.Assume for simplicity that a cache line can hold exactly one element of the matrix.Figure 1shows the number of conﬂicts for S =976and base addresses ranging from 0to 10,000,000.Here,optimal placement reduces the number of conﬂicts with about 59%compared to the worst case.As can be observed,there is some symmetry,which is described next.Proofs can be found in [Noot05].For a pattern with stride S of size W ,the number of conﬂicts is symmetric around the points b j ,given by:b j =2j +t + S 2 − W S 2−1,where t is the smallest integer that makes b 0positive.The range [0,2b j ]does not reach b j +1.So if one examines the base addresses starting from 0,the range ]2b j ,b j +1]contains new information.In this range,there may be an optimal base address that has not yet been discovered.After the range[0,2b0],which is symmetric since b0is its center,has been reﬂected around b1,both the original and its image are reﬂected again around b2,resulting in four copies of the range.After the next symmetry point,we have eight copies,and so on.It can be proven that for the model described in Section2,the number of conﬂicts for a matrix shows a similar symmetric behavior.The symmetry points for an S×S-matrix areb j =2j+t −S22,where t is the smallest integer that makes b 0positive.4ConclusionThe base address of a stride pattern or a matrix pattern has a signiﬁcant impact on the num-ber of conﬂicts,when mapped using a XOR-based hash function.This is the opposite of conventional modulo-based hashing,where the base address has no effect at all on self in-terference.These results offer a new possibility for optimizing the number of conﬂicts. References[Frai85]J.F RAILONG,W.J ALBY,AND J.L ENFANT.XOR-schemes:aﬂexible data organi-zation in parallel memories.In Proceedings of the1985International Conference onParallel Processing,pages276–283,August1985.[Gonz97] A.G ONZÁLEZ,M.V ALERO,N.T OPHAM,AND J.P ARCERISA.Eliminating cache conﬂict misses through XOR-based placement functions.In ICS’97:Proceedings ofthe11th international conference on Supercomputing,pages76–83.ACM Press,1997. [Lam91]M.L AM,E.R OTHBERG,AND M.E.W OLF.The cache performance and opti-mizations of blocked algorithms.In Proceedings of the Fourth International Con-ference on Architectural Support for Programming Languages and Operating Systems,pages63–74,April1991.[Noot05] B.N OOTAERT,H.V ANDIERENDONCK,AND K.D E B OSSCHERE.How patterns in memory references affect the performance of hash functions in cache memories.Technical Report R105.002,Ghent University,March2005.[Rau91] B.R AU.Pseudo-randomly interleaved memory.In Proceedings of the18th Annual International Symposium on Computer Architecture,pages74–83,May1991. [Schl93]M.S CHLANSKER,R.S HAW,AND S.S IVARAMAKRISHNAN.Randomization and associativity in the design of placement-insensitive caches.Technical report,HPComputer Systems Laboratory,June1993.[Toph99]N.T OPHAM AND A.G ONZÁLEZ.Randomized Cache Placement for Eliminating Conﬂicts.IEEE Transactions on Computers,48(2):185–192,1999.[Vand05]H.V ANDIERENDONCK AND K.D E B OSSCHERE.XOR-based Hash Functions.IEEE Transactions on Computers,54(7):800–812,2005.。

dynamic programming 动态规划

Naturally, the TSP can be solved in time On!, by enumerating all tours |but this is very impractical. Since the TSP is one of the NP-complete problems, we have little hope of developing a polynomialamic programming gives an algorithm of complexity On22n |exponential, but much faster than n!.
A B C D with total cost 20 300 10 + 40 20 10 + 40 10 100 = 108; 000. Among
the ve possible orders the ve possible binary trees with four leaves this latter method is
2. Travelling Salesman Problem:
The traveling salesman problem. Suppose that you are given n cities and the distances dij between any two cities; you wish to nd the shortest tour that takes you from your home city to all cities and back.
for all j 2 S; j 6= 1 do
opt:=
mfCinjS6=;1jC :=f1m; 2i;n:i6=: :j;;in2Sg;

SADISA包（版本1.2）：物种丰度分布与独立物种假设说明书

Package‘SADISA’October12,2022Type PackageTitle Species Abundance Distributions with Independent-SpeciesAssumptionVersion1.2Author Rampal S.Etienne&Bart HaegemanMaintainer Rampal S.Etienne<******************>Description Computes the probability of a set of species abundances of a single or multiple sam-ples of individuals with one or more guilds under a mainland-island model.One must spec-ify the mainland(metacommunity)model and the island(local)community model.It as-sumes that speciesﬂuctuate independently.The package also contains functions to simulate un-der this model.See Haegeman,B.&R.S.Etienne(2017).A general sampling formula for com-munity structure data.Methods in Ecology&Evolution8:1506-1519<doi:10.1111/2041-210X.12807>.License GPL-3LazyData FALSERoxygenNote6.1.1Encoding UTF-8Depends R(>=3.5)Imports pracma,DDD(>=4.1)Suggests testthat,knitr,rmarkdown,VignetteBuilder knitrNeedsCompilation noRepository CRANDate/Publication2019-10-2312:10:02UTCR topics documented:convert_fa2sf (2)datasets (2)ﬁtresults (3)12datasets integral_peak (4)SADISA_loglik (5)SADISA_ML (6)SADISA_sim (8)SADISA_test (9)Index11 convert_fa2sf Converts different formats to represent multiple sample dataDescriptionConverts the full abundance matrix into species frequencies If S is the number of species and M is the number of samples,then fa is the full abundance matrix of dimension S by M.The for example fa=[010;321;010]leads to sf=[0102;3211];Usageconvert_fa2sf(fa)Argumentsfa the full abundance matrix with species in rows and samples in columnsValuethe sample frequency matrixReferencesHaegeman,B.&R.S.Etienne(2017).A general sampling formula for community structure data.Methods in Ecology&Evolution.In press.datasets Data sets of various tropical forest communitiesDescriptionVarious tree commnunity abundance data sets to test and illustrate the Independent Species ap-proach.•dset1.abunvec contains a list of6samples of tree abundances from6tropical forest plots(BCI, Korup,Pasoh,Sinharaja,Yasuni,Lambir).•dset2.abunvec contains a list of11lists with one of11samples from BCI combined with samples from Cocoli and Sherman.ﬁtresults3•dset3.abunvec contains a list of6lists with2samples,each from one dispersal guild,for6tropical forest communities(BCI,Korup,Pasoh,Sinharaja,Yasuni,Lambir).•dset4a.abunvec contains a list of6samples from6censuses of BCI(1982,1985,1990,1995,200,2005)with dbh>1cm.•dset4b.abunvec contains a list of6samples from6censuses of BCI(1982,1985,1990,1995,200,2005)with dbh>10cm.Usagedata(datasets)FormatA list of5data sets.See description for information on each of these data sets.Author(s)Rampal S.Etienne&Bart HaegemanSourceCondit et al.(2002).Beta-diversity in tropical forest trees.Science295:666-669.See also11.Janzen,T.,B.Haegeman&R.S.Etienne(2015).A sampling formula for ecological communitieswith multiple dispersal syndromes.Journal of Theoretical Biology387,258-261.fitresults Maximum likelihood estimates and corresponding likelihood valuesfor variousﬁts to various tropical forest communitiesDescriptionMaximum likelihood estimates and corresponding likelihood values for variousﬁts to various trop-ical forest communities,to test and illustrate the Independent Species approach.•ﬁt1a.llikopt contains maximum likelihood values ofﬁt of pm-dl model to dset1.abunvec•ﬁt1a.parsopt contains maximum likelihood parameter estimates ofﬁt of pm-dl model to dset1.abunvec •ﬁt1b.llikopt contains maximum likelihood values ofﬁt of pmc-dl model to dset1.abunvec•ﬁt1b.parsopt contains maximum likelihood parameter estimates ofﬁt of pmc-dl model todset1.abunvec•ﬁt2.llikopt contains maximum likelihood values ofﬁt of rf-dl model to dset1.abunvec•ﬁt2.parsopt contains maximum likelihood parameter estimates ofﬁt of rf-dl model to dset1.abunvec •ﬁt3.llikopt contains maximum likelihood values ofﬁt of dd-dl model to dset1.abunvec•ﬁt3.parsopt contains maximum likelihood parameter estimates ofﬁt of dd-dl model to dset1.abunvec •ﬁt4.llikopt contains maximum likelihood values ofﬁt of pm-dl model to dset2.abunvec(mul-tiple samples)4integral_peak •ﬁt4.parsopt contains maximum likelihood parameter estimates ofﬁt of pm-dl model to dset1.abunvec(multiple samples)•ﬁt5.llikopt contains maximum likelihood values ofﬁt of pm-dl model to dset3.abunvec(mul-tiple guilds)•ﬁt5.parsopt contains maximum likelihood parameter estimates ofﬁt of pm-dl model to dset3.abunvec (multiple guilds)•ﬁt6.llikopt contains maximum likelihood values ofﬁt of pr-dl model to dset1.abunvec•ﬁt6.parsopt contains maximum likelihood parameter estimates ofﬁt of pr-dl model to dset1.abunvec •ﬁt7.llikopt contains maximum likelihood values ofﬁt of pm-dd model to dset1.abunvec•ﬁt7.parsopt contains maximum likelihood parameter estimates ofﬁt of pm-dd model to dset1.abunvec •ﬁt8a.llikopt contains maximum likelihood values ofﬁt of pm-dd model to dset4a.abunvec•ﬁt8a.parsopt contains maximum likelihood parameter estimates ofﬁt of pm-dd model todset4a.abunvec•ﬁt8b.llikopt contains maximum likelihood values ofﬁt of pm-dd model to dset4b.abunvec•ﬁt8b.parsopt contains maximum likelihood parameter estimates ofﬁt of pm-dd model todset4b.abunvecUsagedata(fitresults)FormatA list of20lists,each containing either likelihood values or the corresponding parameter estimates.See description.Author(s)Rampal S.Etienne&Bart HaegemanSourceCondit et al.(2002).Beta-diversity in tropical forest trees.Science295:666-669.integral_peak Computes integral of a very peaked functionDescription#computes the logarithm of the integral of exp(logfun)from0to Inf under the following assump-tions:Usageintegral_peak(logfun,xx=seq(-100,10,2),xcutoff=2,ycutoff=40,ymaxthreshold=1e-12)SADISA_loglik5Argumentslogfun the logarithm of the function to integratexx the initial set of points on which to evaluate the functionxcutoff when the maximum has been found among the xx,this parameter sets the width of the interval toﬁnd the maximum inycutoff set the threshold below which(on a log scale)the function is deemed negligible,i.e.that it does not contribute to the integral)ymaxthreshold sets the deviation allowed inﬁnding the maximum among the xxValuethe result of the integrationReferencesHaegeman,B.&R.S.Etienne(2017).A general sampling formula for community structure data.Methods in Ecology&Evolution.In press.SADISA_loglik Computes loglikelihood for requested modelDescriptionComputes loglikelihood for requested model using independent-species approachUsageSADISA_loglik(abund,pars,model,mult="single")Argumentsabund abundance vector or a list of abundance vectors.When a list is provided and mult=’mg’(the default),it is assumed that the different vectors apply to dif-ferent guilds.When mult=’ms’then the different vectors apply to multiplesamples from the same metacommunity.In this case the vectors should haveequal lengths and may contain zeros because there may be species that occur inmultiple samples and species that do not occur in some of the samples.Whenmult=’both’,abund should be a list of lists,each list representing multiple guildswithin a samplepars a vector of model parameters or a list of vectors of model parameters.Whena list is provided and mult=’mg’(the default),it is assumed that the differentvectors apply to different guilds.Otherwise,it is assumed that they apply tomultiple samples.model the chosen combination of metacommunity model and local community model as a vector,e.g.c(’pm’,’dl’)for a model with point mutation in the metacom-munity and dispersal limitation.The choices for the metacommunity modelare:’pm’(point mutation),’rf’(randomﬁssion),’pr’(protracted speciation),’dd’(density-dependence).The choices for the local community model are:’dl’(dispersal limitation),’dd’(density-dependence).mult When set to’single’(the default),the loglikelihood for a single sample is com-puted When set to’mg’the loglikelihood for multiple guilds is computed.Whenset to’ms’the loglikelihood for multiple samples from the same metacommu-nity is computed.When set to’both’the loglikelihood for multiple guilds withinmultiple samples is computed.DetailsNot all combinations of metacommunity model and local community model have been implemented yet.because this requires checking for numerical stability of the integration.The currently avail-able model combinations are,for a single sample,c(’pm’,’dl’),c(’pm’,’rf’),c(’dd’,’dl’),c(’pr’,’dl’), c(’pm’,’dd’),and for multiple samples,c(’pm’,’dl’).ValueloglikelihoodReferencesHaegeman,B.&R.S.Etienne(2017).A general sampling formula for community structure data.Methods in Ecology&Evolution8:1506-1519.doi:10.1111/2041-210X.12807Examplesdata(datasets);abund_bci<-datasets$dset1.abunvec[[1]];data(fitresults);data.paropt<-fitresults$fit1a.parsopt[[1]];result<-SADISA_loglik(abund=abund_bci,pars=data.paropt,model=c( pm , dl ));cat( The difference between result and the value in fitresults.RData is: ,result-fitresults$fit1a.llikopt[[1]]);SADISA_ML Performs maximum likelihood parameter estimation for requestedmodelDescriptionComputes maximum loglikelihood and corresponding parameters for the requested model using the independent-species approach.For optimization it uses various auxiliary functions in the DDD package.UsageSADISA_ML(abund,initpars,idpars,labelpars,model=c("pm","dl"),mult="single",tol=c(1e-06,1e-06,1e-06),maxiter=min(1000*round((1.25)^sum(idpars)),1e+05),optimmethod="subplex",num_cycles=1)Argumentsabund abundance vector or a list of abundance vectors.When a list is provided and mult=’mg’(the default),it is assumed that the different vectors apply to dif-ferent guilds.When mult=’ms’then the different vectors apply to multiplesamples.from the same metacommunity.In this case the vectors should haveequal lengths and may contain zeros because there may be species that occur inmultiple samples and species that do not occur in some of the samples.initpars a vector of initial values of the parameters to be optimized andﬁxed.See labelpars for more explanation.idpars a vector stating whether the parameters in initpars should be optimized(1)or remainﬁxed(0).labelpars a vector,a list of vectors or a list of lists of vectors indicating the labels integers (starting at1)of the parameters to be optimized andﬁxed.These integers cor-respond to the position in initpars and idpars.The order of the labels in thevector/list isﬁrst the metacommunity parameters(theta,and phi(for protractedspeciation)or alpha(for density-dependence or abundance-dependent specia-tion)),then the dispersal parameters(I).See the example and the vignette formore explanation.model the chosen combination of metacommunity model and local community model as a vector,e.g.c(’pm’,’dl’)for a model with point mutation in the metacom-munity and dispersal limitation.The choices for the metacommunity modelare:’pm’(point mutation),’rf’(randomﬁssion),’pr’(protracted speciation),’dd’(density-dependence).The choices for the local community model are:’dl’(dispersal limitation),’dd’(density-dependence).mult When set to’single’(the default),the loglikelihood for a single sample and single guild is computed.When set to’mg’,the loglikelihood for multiple guildsis computed.When set to’ms’the loglikelihood for multiple samples from thesame metacommunity is computed.tol a vector containing three numbers for the relative tolerance in the parameters,the relative tolerance in the function,and the absolute tolerance in the parameters.maxiter sets the maximum number of iterationsoptimmethod sets the optimization method to be used,either subplex(default)or an alternative implementation of simplex.num_cycles the number of cycles of opimization.If set at Inf,it will do as many cycles as needed to meet the tolerance set for the target function.8SADISA_simDetailsNot all combinations of metacommunity model and local community model have been implemented yet.because this requires checking for numerical stability of the integration.The currently avail-able model combinations are,for a single sample,c(’pm’,’dl’),c(’pm’,’rf’),c(’dd’,’dl’),c(’pr’,’dl’), c(’pm’,’dd’),and for multiple samples,c(’pm’,’dl’).ReferencesHaegeman,B.&R.S.Etienne(2017).A general sampling formula for community structure data.Methods in Ecology&Evolution8:1506-1519.doi:10.1111/2041-210X.12807Examplesutils::data(datasets);utils::data(fitresults);result<-SADISA_ML(abund=datasets$dset1.abunvec[[1]],initpars=fitresults$fit1a.parsopt[[1]],idpars=c(1,1),labelpars=c(1,2),model=c( pm , dl ),tol=c(1E-1,1E-1,1E-1));#Note that tolerances should be set much lower than1E-1to get the best results. SADISA_sim Simulates species abundance dataDescriptionSimulates species abundance data using the independent-species approachUsageSADISA_sim(parsmc,ii,jj,model=c("pm","dl"),mult="single",nsim=1)Argumentsparsmc The model parameters.For the point mutation(pm)model this is theta and I.For the protracted model(pr)this is theta,phi and I.For the density-dependentmodel(dd)-which can also be interpreted as the per-species speciation model,this is theta and alpha.ii The I parameter.When I is a vector,it is assumed that each value describes a sample or a guild depending on whether mult==’ms’or mult==’mg’.Whenmult=’both’,a list of lists must be speciﬁed,with each list element relates to asample and contains a list of values across guilds.jj the sample sizes for each sample and each guild.Must have the same structure as iimodel the chosen combination of metacommunity model and local community model as a vector,e.g.c(’pm’,’dl’)for a model with point mutation in the metacom-munity and dispersal limitation.The choices for the metacommunity modelare:’pm’(point mutation),’rf’(randomﬁssion),’pr’(protracted speciation),’dd’(density-dependence).The choices for the local community model are:’dl’(dispersal limitation),’dd’(density-dependence).mult When set to’single’,the loglikelihood of a single abundance vector will be com-puted When set to’mg’the loglikelihood for multiple guilds is computed.Whenset to’ms’the loglikelihood for multiple samples from the same metacommu-nity is computed.When set to’both’the loglikelihood for multiple guilds withinmultiple samples is computed.nsim Number of simulations to performDetailsNot all combinations of metacommunity model and local community model have been implemented yet.because this requires checking for numerical stability of the integration.The currently available model combinations are c(’pm’,’dl’).Valueabund abundance vector,a list of abundance vectors,or a list of lists of abundance vectors,or a list of lists of lists of abundance vectors Theﬁrst layer of the lists corresponds to different simulations When mult=’mg’,each list contains a list of abundance vectors for different guilds.When mult =’ms’,each list contains a list of abundance vectors for different samples from the same meta-community.In this case the vectors should have equal lengths and may contain zeros because there may be species that occur in multiple samples and species that do not occur in some of the samples.When mult=’both’,each list will be a list of lists of multiple guilds within a sampleReferencesHaegeman,B.&R.S.Etienne(2017).A general sampling formula for community structure data.Methods in Ecology&Evolution8:1506-1519.doi:10.1111/2041-210X.12807SADISA_test Tests SADISA for data sets included in the paper by Haegeman&Eti-enneDescriptionTests SADISA for data sets included in the paper by Haegeman&EtienneUsageSADISA_test(tol=0.001)Argumentstol tolerance of the testReferencesHaegeman,B.&R.S.Etienne(2017).A general sampling formula for community structure data.Methods in Ecology&Evolution.In press.Index∗datasetsdatasets,2fitresults,3∗modelSADISA_loglik,5SADISA_ML,6SADISA_sim,8SADISA_test,9∗species-abundance-distributionSADISA_loglik,5SADISA_ML,6SADISA_sim,8SADISA_test,9convert_fa2sf,2datasets,2fitresults,3integral_peak,4SADISA_loglik,5SADISA_ML,6SADISA_sim,8SADISA_test,911。

小学上册A卷英语自测题(有答案)

小学上册英语自测题(有答案)英语试题一、综合题(本题有100小题，每小题1分，共100分.每小题不选、错误，均不给分)1.What is the capital of Malta?A. VallettaB. MdinaC. GozoD. Rabat答案:A2.The _____ (商场) is open all day.3.历史上，________ (empires) 的兴衰与经济息息相关。

4.The ancient Greeks believed in many ________.5.I like to participate in ______ (社区活动) to meet new people.6.Many animals use __________ to find their way home.7.In chemistry, a solvent is usually present in a _____ (greater amount) than the solute.8.The wind is ___ (strong/light).9. A __________ is a city that is not the capital but is important for trade.10.I like to bake ______ on weekends.11.The flowers brighten up the _______ (花使_______变得明亮).12.The _____ (生长) of a plant depends on sunlight and water.13.The boy has a new ________.14.The first successful vaccine was for _______. (天花)15.The goldfish swims in circles in its _________ (鱼缸).16.The __________ (历史的教学) guides our learning.17.The ________ is a popular choice for pets.18.What sound does a dog make?A. MeowB. MooC. BarkD. Quack答案:C19.The country famous for its lakes is ________ (加拿大).20.The fish swims _____ (fast/slow) in the water.21.The ________ (forum) facilitates discussion.22.The ______ is a great cook.23.The process of electrolysis uses electricity to drive a ______.24.I feel proud when my parents call me ______. (当我的父母称我为____时，我感到自豪。

关于连通度的无三圈图的划分

㊀R e c e i v e dd a t e :２０１８Ｇ０７Ｇ０１;㊀M o d i f i e dd a t e :２０１８Ｇ０９Ｇ０５㊀F u n d p r o j e c t s :N a t u r a l S c i e n c eF o u n d a t i o no f J i a n g s uP r o v i n c e (B K ２０１７０８６２);N S F C (１１７０１１４２)㊀A u t h o r i n t r o d u c t i o n :L IR u i (１９８２－),m a l e ,D o c t o r o fM a t h e m a t i c s ,m a j o r i n g r a p h t h e o r y．E m a i l :l i r u i ＠h h u ．e d u ．c n 第３４卷第５期大㊀学㊀数㊀学V o l ．３４,ɴ．５２０１８年１０月C O L L E G E MA T H E MA T I C S O c t ．２０１８P a r t i t i o n s o fT r i a n g l e Ｇf r e eG r a p h sw i t h R e s t r i c t i o no nC o n n e c t i v i t yL IR u i (D e p a r t m e n t o fM a t h e m a t i c s ,C o l l e g e o f S c i e n c e s ,H o h a iU n i v e r s i t y ,N a n j i n g ２１１１００,C h i n a )㊀㊀A b s t r a c t :K üh na n dO s t h u s p r o v e d t h a t f o r e v e r yp o s i t i v e i n t e g e r l ,t h e r e e x i s t s a n i n t e g e r k (l )ɤ２１６l ２,s u c h t h a t t h ev e r t e xs e to fe v e r y k (l )Ｇc o n n e c t e d g r a p h s G c a nb e p a r t i t i o n e di n t os u b s e t s S a n d T w i t ht h e p r o p e r t i e s t h a t b o t h G [S ]a n d G [T ]a r e l Ｇc o n n e c t e d a n d e v e r y v e r t e x i n S h a s a t l e a s t l n e i g h b o r s i n T ．I n t h i s p a p e r ,w e c o n s i d e r t h eu p p e rb o u n do f k (l )o nt r i a n g l e Ｇf r e e g r a p h s ．A f t e rs h o w i n g t h a te v e r y t r i a n gl e Ｇf r e e g r a p ho f a v e r a g ed e g r e ea t l e a s t ８l /３h a sa n l Ｇc o n n e c t e ds u b g r a ph ,w e p r o v et h a t k (l )ɤ２１６３－３ l ２o n t r i a n g l e Ｇf r e e g r a p h s ．K e y wo r d s :c o m b i n a t o r i a l p r o b l e m s ;g r a p h p a r t i t i o n s ;c o n n e c t i v i t y ;t r i a n g l e Ｇf r e e C L CN u m b e r :O １５７．５㊀㊀D o c u m e n t C o d e :A㊀㊀A r t i c l e I D :１６７２Ｇ１４５４(２０１８)０５Ｇ０００１Ｇ０６１㊀I n t r o d u c t i o nA l l g r a p h s c o n s i d e r e d i n t h i s p a p e r a r e f i n i t e ,u n d i r e c t e d a n d s i m p l e ．F o r a g r a p h ,w e d e n o t eb y V (G ),E (G ),d (G )a n d δ(G )t h e v e r t e x s e t ,e d g e s e t ,a v e r a g e d e g r e e a n d t h em i n i m u md e gr e e o f G ,r e s p e c t i v e l y ．W eu s e |G |a n d e (G ),r e s p e c t i v e l y ,t od e n o t e t h en u m b e r so f v e r t i c e s a n de d g e s i na g r a p h G ．F o r a s u b s e t S ⊆V (G ),w eu s e G [S ]t od e n o t e t h e s u b g r a p ho f G i n d u c e db y S ,a n du s e e S ()t od e n o t et h en u m b e ro fe d g e s i n G [S ]．F o rt w od i s j o i n ts u b s e t s S a n d T o f V (G ),w eu s e e (S ,T )t od e n o t e t h en u m b e r o f e d ge sw i t ho n e e n d i n S a n d t h e o t h e r i n T ．G i v e na g r a p h G ,a p a r t i t i o nof G i s a f a m i l y o f p a i r w i s ed i s j o i n t s u b s e t s V １, ,V k o f V (G )s u c h t h a t V (G )＝ɣk i ＝１．G r a p h p a r t i t i o n p r o b l e m sa s kf o ra p a r t i t i o no f t h ev e r t e xs e to fag r a ph wi t h v a r i o u s r e q u i r e m e n t s ．O n em a y a s k t h e s m a l l e s t n u m b e r k o f p a r t i t i o n i n g V (G )i n t o k s u b s e t s o fw h i c h e a c h i n d u c e s a p a t h (T h i s i sc a l l e dt h e p a t h p a r t i t i o n p r o b l e m．T h e r ea r e l i n e a r Ｇt i m ea l g o r i t h m s f o r s o m e s p e c i a l f a m i l y o f g r a p h s [１,１５],b u t i t i s N P Ｇc o m p l e t ei n g e n e r a l )．T h e m a x i m a lb a l a n c e d c o n n e c t e d p a r t i t i o n p r o b l e m a s k sf o ra p a r t i t i o n o f V (G )i n t ot w os u b s e t s V １,V ２e a c hi n d u c e sa c o n n e c t e d s u b g r a p h s a n dm a x i m i z e sm i n {V １,V ２}(i t s a p p r o x i m a b i l i t y w a s s t u d i e d i n [３])．T h e m a x i m u mb i p a r t i t es u b g r a p h p r o b l e m a s k sf o rab i p a r t i t i o no f V (G )t h a tm a x i m i z e st h en u m b e ro f e d ge sb e t w e e n t h e t w o s u b s e t s (r e a d e r s a r e r ef e r r e d t o [１０]f o rd e t a i l s )．Al o t o f o t h e r p r o b l e m s i ng r a ph t h e o r y c a na l s ob e p r e s e n t e da s g r a p h p a r ti t i o n s ．F o r i n s t a n c e ,v a r i o u s c o l o r i n gp r o b l e m s a s k s f o r p a r t i t i o n so f V (G )i n t oi n d e pe n d e n ts e t s w i t hs o m ef u r t h e rr e s t r i c t i o n s (s e e [２,８,１４,１６]f o r e x a m p l e s )．２大㊀学㊀数㊀学㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀第３４卷H e r ew e c o n c e r nw i t ha p a r t i t i o n p r o b l e mt h a t a s k s f o ra p a r t i t i o no f V(G)i n t ot w os u b s e t so f w h i c he a c h i n d u c e s a s u b g r a p hw i t h p r e s c r i b e dc o n n e c t i v i t y(r e s p．m i n i m u md e g r e e)．G yör i p o s e da p r o b l e mi n１９８１:f o r e a c h p a i r s,t o f p o s i t i v e i n t e g e r s,d o e s t h e r e e x i s t a p o s i t i v e i n t e g e r s g(s,t)s u c h t h a t t h e v e r t e x s e t o f e a c h g r a p h o f c o n n e c t i v i t y a t l e a s t g(s,t)c a nb e p a r t i t i o n e d i n t o t w o s e t s S a n d T w h i c h i n d u c e s u b g r a p h s o f c o n n e c t i v i t y a t l e a s t S a n d T,r e s p e c t i v e l y(s e e[５,１３])．T h o m a s s e n[１３], a n d i n d e p e n d e n t l y S z e g e d y[５]e s t a b l i s h e d t h e e x i s t e n c e o f t h e f u n c t i o n g(s,t)．T h o m a s s e n s p r o o f i sb a s e do nar e s u l to fM a d e r[９]c l a i m i n g t h a t s u f f i c i e n t l y l a r g em i n i m u m d e g r e ew i l l g u a r a n t e e t h ee x i s t e n c eo fh i g h l y c o n n e c t e ds u b g r a p h s．H es h o w e dt h a t f o re a c h p a i ro f p o s i t i v e i n t e g e r s S a n d T,t h e r ee x i s t sa f u n c t i o n f(s,t)s u c ht h a t t h ev e r t e xs e to f a n yg r a p h w i t h m i n i m u md e g r e e a t l e a s t f(s,t)h a sa p a r t i t i o n S,T w h i c h i n d u c es u b g r a p h so fm i n i m u m d e g r e ea t l e a s t s a n d t,r e s p e c t i v e l y,a n dh e f u r t h e r c o n j e c t u r e d t h a t f(s,t)＝s＋t＋１．I n１９８３,H a j n a l[５] p r e s e n t e du p p e rb o u n d sf o rb o t h f(s,t)a n d g(s,t)．T h o m a s s e n sc o n j e c t u r e w a sc o n f i r m e d b y S t i e b i t z[１１]i n１９９６．T h e o r e m１．１(S t i e b i t z,[１１])㊀L e t G b e a g r a p h a n d s,tȡ０b e i n t e g e r s．I fδ(G)ȡs＋t＋１,t h e n t h e r e i s a p a r t i t i o n S,T o f V(G)s u c h t h a tδ(G[S])ȡs a n dδ(G[T])ȡt．K s＋t＋１s h o w s t h a t o n e c a n n o t d e c r e a s e t h em i n i m u md e g r e e f u r t h e rm o r e．L e t S,T b ea p a r t i t i o no fa g r a p h G．W eu s e(S,T)G t od e n o t et h eb i p a r t i t es u b g r a p ho f G c o n s i s t i n g o f t h e e d g e s b e t w e e n S a n d T．I t i sw e l l k n o w nt h a t t h em i n i m u md e g r e eo f t h eb i p a r t i t e s u b g r a p h(S,T)G o f G c a nb e g r e a t e rt h a nh a l ft h e m i n i m u m d e g r e eo f G．S oi t i sa ni n t e r e s t i n g q u e s t i o n t o s e e t h a tw h e t h e r G[S],G[T]a n d(S,T)G c a nh a v e l a r g em i n i m u md e g r e e o r c o n n e c t i v i t y s i m u l t a n e o u s l y．I n[６],Küh n a n dO s t h u s g a v e a n e g a t i v e a n s w e r t o t h i s q u e s t i o nb y s h o w i n g t h a t f o r e a c h p o s i t i v e i n t e g e r k,t h e r e e x i s t s a kＧc o n n e c t e d g r a p h G w h o s e v e r t e x s e t c a n n o t b e p a r t i t i o n e d i n t o n o n e m p t y s e t s S a n d T s u c h t h a t e a c hv e r t e xo f G h a s n e i g h b o r s i nb o t h S a n d T．T h e n i t i s w o r t hc o n s i d e r i n g w h e t h e ro n es i d eo f(S,T)G c a nh a v el a r g e m i n i m u m d e g r e eo r c o n n e c t i v i t y w h i l e t h e m i n i m u m d e g r e ea n dc o n n e c t i v i t y o f G[S],G[T]a r eb o t hl a r g e．Küh na n d O s t h u s a n s w e r e di ta f f i r m a t i v e l y[６]．T h e y p r o v e dt h a tf o re a c h p o s i t i v ei n t e g e r l,t h e r ee x i s t i n t e g e r s kᶄ(l)ɤ２１１３l２a n d k(l)ɤ２１６l２,s u c ht h a tδ(G)ȡkᶄ(l)g u a r a n t e e st h ee x i s t e n c eo fa p a r t i t i o no f V(G)i n t o S a n d T s u c h t h a tδ(G[S]),δ(G[T])ȡ１a n d e v e r y v e r t e xo f S h a sa t l e a s t l n e i g hb o r s i n T,a n dc o n n e c t i v i t y a t l e a s t k(l)g u a r a n t e e st h e e x i s t e n c e o f a p a r t i t i o no f V(G)i n t o Sᶄa nd Tᶄs u c ht h a tb o t h G[Sᶄ]a n d G[Tᶄ]a re lＧc o n n e c t e da n d e v e r y v e r t e xof Sᶄh a s a t l e a s t l n e igh b o r si n Tᶄ．I n t h i s p a p e r,w e f o c u so nt r i a n g l eＧf r e e g r a p h s,c o n s i d e r t h e p a r t i t i o n p r o b l e m sw i t hr e s t r i c t i o n o n c o n n e c t i v i t y,a n d p r o v e t h e f o l l o w i n g t h e o r e m．T h e o r e m１．２㊀F o r g i v e n p o s i t i v e i n t e g e r s sȡt,t h ev e r t e xs e to fe v e r y２１６３－３l２Ｇc o n n e c t e d t r i a n g l eＧf r e e g r a p h G c a nb e p a r t i t i o n e di n t on o n e m p t y s e t s S a n d T s u c ht h a t G[S]a n d G[T]a r e sＧc o n n e c t e d a n d tＧc o n n e c t e d,r e s p e c t i v e l y,a n d e v e r y v e r t e x i n S h a s a t l e a s t t n e i g h b o r s i n T．B y t a k i n g s＝t＝１,w e s e e t h a t k(l)ɤ２１６３－３l２o n t r i a n g l eＧf r e e g r a p h s．T h e p r o o f t e c h n i q u e o fT h e o r e m１．２i s s i m i l a r t o t h a t u s e db y Küh na n dO s t h u s．２㊀T r i a n g l eＧf r e e g r a p h sI n t h i s s e c t i o n,w e f o c u so nt r i a n g l eＧf r e e g r a p h s,c o n s i d e r i t s p a r t i t i o n p r o b l e m w i t hr e s t r i c t i o n o n c o n n e c t i v i t y,a n d p r o v eT h e o r e m１．２．O u r p r o o f s a r e a l s ob a s e do na t h e o r e mo fM a d e r．I n１９７２,M a d e r p r o v e d t h e f o l l o w i n g r e s u l t t h a t g i v e s a s u f f i c i e n t c o n d i t i o n f o r a g r a p h c o n t a i n i n ga k Ｇc o n n e c t e d s ub g r a p h ．T h e o r e m２．１(M a d e r ,[９])㊀L e t k ȡ１a n d μȡ－１b e i n t e g e r s ,a n d l e t G b ea g r a p hs uc ht h a t |G |ȡk ＋μ＋２a nde (G )＞k ＋μ()|G |－k ＋１(),a n d s u c h t h a t e H ()ɤk ＋μ()H －k ＋１()f o r e a c hs u bg r a ph H o f G wi t h k ＋μ＋２v e r t i c e s ．T h e n ,G h a s a k Ｇc o n n e c t e d s u b g r a p h ．A s ad i r e c t c o n s e q u e n c e o fT h e o r e m２．１(b y t a k i n g μ＝k －３),f o r g i v e n i n t e g e r k ȡ１,e v e r y g r a p hw i t ha v e r a g e d e g r e e a t l e a s t ４k －６h a s a k Ｇc o n n e c t e ds u b g r a p h (s e eT h e o r e m１．４．２o f [４])．T h i s b o u n d i s s h a r p a se v i d e n c e db y t h ec y c l e s ．B u to nt r i a n g l e Ｇf r e e g r a p h s ,t h ea v e r a g ed e g r e e f o r g u a r a n t e e i n g t h e e x i s t e n c eo f k Ｇc o n n e c t e ds u b g r a p h sm a y b er e d u c e dt o ８k /３．T ob e p r e c i s e l y ,w e h a v e t h e f o l l o w i n g T h e o r e m２．２㊀L e t G b ea t r i a n g l e Ｇf r e e g r a p h ,a n d k b ea p o s i t i v e i n t e g e r ．I f d (G )ȡ８k /３－２,t h e n G c o n t a i n s a k Ｇc o n n e c t e d s u b g r a p h ．P r o o f ㊀W h i l e k ＝１o r ２,t h e c o n c l u s i o nh o l d s t r i v i a l l y ．S o ,w e s u p p o s e t h a t k ȡ３,a n da p p l y T h e o r e m１．２w i t h μ＝k /３－１．S i n c e d (G )ȡ８k /３－２,|G |ȡ８k /３－１＞４k /３＋１＝k ＋μ＋２,a n d e (G )ȡ８k /３－２()|G |/２＝４k /３－１()|G |＞k ＋μ()|G |－k ＋１()．L e t H b e a s u b g r a p ho f G w i t h H ＝k ＋μ＋２．S i n c e G i s t r i a n gl e Ｇf r e e ,e H ()ɤ１４k ＋μ＋２()２＝４k ２/９＋２k /３＋１/４ɤ４k ２/９＋７k /３－２＝k ＋μ()H －k ＋１()．B y T h e o r e m２．１,G c o n t a i n s a k Ｇc o n n e c t e d s u b g r a p h ．T h e p r o o f i s c o m p l e t e ．W h i l e t a k i n g k ＝１,８k /３－２＝１．S i n c e δ(G )ȡ１i s a n e c e s s a r y c o n d i t i o n f o r G c o n t a i n i n g a n o n t r i v i a l c o n n e c t e d s u b g r a p h ,t h eb o u n d i nT h e o r e m２．２i s a l m o s t s h a r pi n t h i s s e n s e ．T h o m a s s e n p r o v e d [１３]t h a t ,f o r e a c h p a i r s ,t o f p o s i t i v e i n t e g e r s ,t h e r e e x i s t s a n i n t e g e r f s ,t ()s u c ht h a t e a c h s ＋t －１Ｇc o n n e c t e d g r a p hw i t hm i n i m u md e g r e e a t l e a s t f s ,t ()h a s a p a r t i t i o n i n t o t w o s e t sw h i c h i n d u c e s u b g r a p h s o f c o n n e c t i v i t y a t l e a s t s a n d t ,r e s p e c t i v e l y ．C o m b i n i n g T h e o r e m１．１a n d T h e o r e m２．２,w e g i v ea m i n i m u m d e g r e ec o n d i t i o nt od e a lw i t ht r i a n g l e Ｇf r e e g r a p h s ．T h e p r o o f t e c h n i q u e i s t h e s a m e a s t h a t u s e db y Th o m a s s e n i n [１３]．T h e o r e m２．３㊀I f G i s a n s ＋t －１Ｇc o n n e c t e d t r i a n g l e Ｇf r e e g r a p hw i t h δ(G )ȡ８/３s ＋t ()－２,t h e n t h e r e e x i s t s a p a r t i t i o n S ,T o f V (G )s u c h t h a t G [S ]a n d G [T ]a r e s Ｇc o n n e c t e da n d t Ｇc o n n e c t e d ,r e s p e c t i v e l y．P r o o f ㊀S i n c e (８s /３－２)＋８t /３－２()＋１ɤ８s ＋t ()/３－２f o r a n yp o s i t i v e i n t e g e r s s a n d t ,b y T h e o r e m１．１,w em a y o b t a i n a p a r t i t i o n S ᶄ,T ᶄo f V (G )s u c h t h a t δG S ᶄ[]()ȡ８s /３－２a n d δG S ᶄ[]()ȡ８t /３－２．B y T h e o r e m２．２,S ᶄc o n t a i n s a s u b s e t S s u c h t h a t G [S ]i s s Ｇc o n n e c t e d ,a n d T ᶄc o n t a i n s a s u b s e t T s u c h t h a t G [T ]i s t Ｇc o n n e c t e d ．C h o o s e S a n d T s a t i s f y i n g t h o s e p r o p e r t i e ss u c ht h a t S ɣT i s m a x i m u m．W e s h o wt h a t S ɣT ＝V (G )．S u p p o s e t h a t i t i s n o t t h e c a s e ,i ．e ．,S ɣT ʂV (G )．W e s e t C ＝V (G )\(S ɣT )．T h e n ,C ʂ∅．B y t h e c h o i c e o f S ,G S ɣC []i s n o t s Ｇc o n n e c t e d ．F o r o t h e r w i s e ,w eh a v e (S ɣC )ɣT ＞S ɣTc o n t r ad i c t i n g t h em a x i m a l i t y o f S ɣT ．S o G S ɣC []c o n t a i n s a c u t Ｇse t A s u c h t h a t |A |ɤs －１．S i n c e G [S ]i s s Ｇc o n n e c t e d ,t h e r e e x i s t s a c o m p o n e n t H of G [(S ɣC )]\A s u c h t h a t V (H )＝S \A ．S i m i l a r l y ,G T ɣC []i s n o t t Ｇc o n n e c t e d ,a n d i t c o n t a i n s a c u t Ｇs e t B s u c h t h a t B ɤt －１．T h e r e a l s o e x i s t s a c o m p o n e n t s H ᶄo f G [T ɣC ]\B s u c h t h a t V (H ᶄ)＝T \B ．I t i s e a s y t o v e r i f y t h a t H a n d H ᶄa r e ３第５期㊀㊀㊀L IR u i :P a r t i t i o n s o fT r i a n g l e Ｇf r e eG r a p h sw i t hR e s t r i c t i o no nC o n n e c t i v i t ys e p a r a t e db y A ɣB i n g r a p h G ．T h i s i m p l i e s t h a t A ɣB i s a c u t Ｇs e t o f G ．B u t |A ɣB |ɤs ＋t －２,c o n t r a d i c t i n g t h e s ＋t －１()Ｇc o n n e c t i v i t y of G ．B e f o r e p r o v i ng Th e o r e m１．２,w e s ti l l n e e d t h e f o l l o w i n g l e m m a c o n c e r n i n g w i t h t h e p a r t i t i o n s o f t r i a n g l e Ｇf r e e g r a p h s i n t o t w o s u b s e t s o fw h i c ho n e i n d u c e s a s u b g r a p hw i t hh i g h c o n n e c t i v i t y ,a n d t h e o t h e r i n d u c e s a s u b g r a p hw i t hn o t o n l y h i g hc o n n e c t i v i t y b u t a l s o l o w e r a v e r a g e d e g r e e ．L e m m a２．４㊀F o r g i v e n p o s i t i v e i n t e g e r s s ȡt ,t h ev e r t e xs e to f e v e r y １６s /３＋t ()Ｇc o n n e c t e d t r i a n g l e Ｇf r e e g r a p h G c a nb e p a r t i t i o n e d i n t on o n Ｇe m p t y s e t s S a n d T s u c ht h a t G [S ]i s s Ｇc o n n e c t e d ,G [T ]i s t Ｇc o n n e c t e d ,a n d e v e r y s u b g r a p ho f G [S ]h a s a v e r a g e d e g r e e l e s s t h a n １２８s /９．P r o o f ㊀B y T h e o r e m２．３,V (G )c a nb e p a r t i t i o n e d i n t on o n Ｇe m p t y s e t s S a n d T s u c ht h a t G [S ]i s s Ｇc o n n e c t e d ,G [T ]i s t Ｇc o n n e c t e d ,r e s p e c t i v e l y ．C h o o s e S a n d T s a t i s f y i n g t h e p r o p e r t i e s s u c h t h a t S i s m i n i m a l ．W ew i l l s h o wt h a t S a n d T a r e a s d e s i r e d ．S i n c e G [T ]i s t r i a n g l e Ｇf r e ea n d δ(G [T ])ȡt ,f o r t h e t w oe n dv e r t i c e so f e v e r y e d g e i n E (G ),t h e i r n e i g h b o r s h a v en ov e r t e x i n c o m m o n ,h e n c ew e h a v e T ȡ２t ．T h e s a m e a r gu m e n t s h o w s t h a t S ȡ２s ȡ２t ．F i r s tw e c l a i mt h a t G [S ]i sn o t １６s /３Ｇc o n n e c t e d ．F o ro t h e r w i s e ,S c a nb e p a r t i t i o n e d i n t ot w o n o n Ｇe m p t y s e t s S １a n d S ２,b y T h e o r e m２．３,s u c h t h a t e a c h G S i []i s s Ｇc o n n e c t e d ,a n dh e n c e S i ȡ２s ȡ２t ．S i n c e G i s ４t Ｇc o n n e c t e d ,t h e r e a r e a t l e a s t ２t i n d e p e n d e n t e d g e sb e t w e e n S a n d T i n G ．S ow e m a y a s s u m e t h a t S ２c o n t a i n sa t l e a s t t e n d v e r t i c e so f t h e s ee d g e s ．W es e et h a t G S ２ɣT []i sa l s o t Ｇc o n n e c t e d ,t h e n t h e p a r t i t i o n S １,S ２ɣT c o n t r a d i c t s t h em i n i m a l i t y o f S ．N o w w e s h o wt h a t e v e r y s u b g r a p ho f G [S ]i sa v e r a g ed e g r e e l e s s t h a n １２８s /９．S u p p o s e t ot h e c o n t r a r y t h a t G [S ]h a s a s u b g r a p ho f a v e r a g e d e g r e e a t l e a s t １２８s /９．B y T h e o r e m２．２,G [S ]c o n t a i n s a １６s /３Ｇc o n n e c t e d s u b g r a p h ,s a y H ．S i n c e G [S ]i s n o t １６s /３Ｇc o n n e c t e d ,t h e r e i s a c u t Ｇs e t X o f G [S ]w i t h X ɤ１６s /３．L e t C b e t h e c o m p o n e n t o f G [S ]－X s u c h t h a t V H ()⊆X ɣV C ()．L e t S ᶄ＝C ɣX ,㊀T ᶄ＝V (G )\S ᶄ．T h e n ,S ᶄi s a p r o p e r s u b s e t o f S ．W e f u r t h e r c l a i mt h a t G [T ᶄ]i s t Ｇc o n n e c t e d ．I f i t i sn o t t h ec a s e ,t h e r em u s t e x i s tac u t Ｇs e t Y o f G [T ᶄ]w i t h Y ＜t ．B e c a u s e G [T ]i s t Ｇc o n n e c t e d ,s ot h e r eh a sa u n i q u e c o m p o n e n t D o f G [T ᶄ]－Y s u c h t h a t T ⊆D ɣY ．S e t F ＝T ᶄ\D ɣY ()．T h e n t h e r e i s n o p a t h f r o m F t o C i n G －X ɣY ()．I t f o l l o w s t h a t X ɣY i sac u t Ｇs e to f G w i t h |X ɣY |＜１６s /３＋t ,c o n t r a d i c t i n g t h a t G i s (１６s /３＋t )Ｇc o n n e c t e d ．S i n c e G [T ᶄ]i s t Ｇc o n n e c t e d ,w em a y s u c c e s s i v e l y m o v e v e r t i c e s f r o m S ᶄt o T ᶄi f t h e y h a v e l e s s t h a n １６s /３n e i g h b o r s i nt h es u b s e to f G [S ᶄ]a l r e a d y c o n s t r u c t e d (n o t et h a t t h e s ev e r t i c e sh a v ea t l e a s t t n e i g h b o r s i n t h e s u p e r s e t o f T ᶄw h i c h p r e s e r v e s t Ｇc o n n e c t e d n e s so f T ᶄ)．B y th e c h o i c eo f S ᶄ,G [S ᶄ]c o n t a i n s H a s a s u b g r a p h ,a n dh e n c e t h e p r o c e s sm u s t t e r m i n a t e a t s o m e s u b g r a p h H ᶄo f G [S ᶄ]w i t h m i n i m u md e g r e e a t l e a s t １６s /３．T h e n ,t h e p a r t i t i o n V H ᶄ(),V (G )\V H ᶄ()c o n t r a d i c t s t h em i n i m a l i t yo f S ．T h e r e f o r e ,e v e r y s u b g r a p ho f G [S ]h a sa v e r a g ed e g r e e l e s s t h a n １２８s /９．T h i sc o m p l e t e s t h e p r o o f ．T h e r e s u l t s o fK ün do s t h u s a r eb a s e do na t e c h n i q u e l e m m a (L e m m a１１o f [６])．B e l o w L e m m a ２．５i sar e v i s i o n o f L e m m a１１o f [６]．B y as l i g h t l y d e t a i l e dc a l c u l a t i o n ,w e m a y de d u c et h e r e q u i r e m e n t o n t h em i n i m u md e g r e e of G f r o m２６c l r t o ２３c l r ．L e m m a ２．５㊀L e t c ȡ２b e a r e a l ,k ,l ,r b e p o s i t i v e i n t eg e r s s u ch t h a t l ȡr a n d k ȡ２３c l r ．L e t G b e a g r a p ho fmi n i m u m d e g r e ea t l e a s t k a n dl e t S ,T b ea p a r t i t i o no f V (G )s u c ht h a t d (G [S ])ȡl ,δ(G [S ])ȡr a n de v e r y s u b g r a p ho f G [S ]h a s a v e r a g ed e gr e e l e s s t h a n c l ．T h e nt h e r ee x i s t s S ᶄ⊆S s u c h t h a t ,w r i t i n g T ᶄ＝V (G )\S ᶄ,e v e r y v e r t e x i n S ᶄh a s a t l e a s t r n e i g h b o r s i n T ᶄ,d (G [S ᶄ])ȡl /８a n d δ(G [T ᶄ])ȡr ．M o r e o v e r ,T ᶄc a nb eo b t a i n e df r o m T b y s u c c e s s i v e l y a d d i n g v e r t i c e sh a v i n g a t l e a s t r n e i g h b o r s i n t h e s u p e r s e t o f T a l r e a d y c o n s t r u c t e d ．４大㊀学㊀数㊀学㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀第３４卷N o w ,w e a r e r e a d y t o p r o v eT h e o r e m１．２．P r o o f o f T h e o r e m１．２㊀S i n c e ８/３６４s /３＋t ()＜３－３２１６s t ,s ob y T h e o r e m２．３,w em a yp a r t i t i o n V (G )i n t o S ᵡ,T ᵡs u c h t h a t G S ᵡ[]i s ６４s /３Ｇc o n n e c t e d ,G T ᵡ[]i s t Ｇc o n n e c t e d a n de v e r y s u b g r a p ho f G S ᵡ[]h a sa v e r a g ed e g r e e l e s st h a n１２８/９６４s /３．N o w a p p l y L e m m a ２．５w i t h p a r a m e t e r s l ＝６４s /３,r ＝t a n d c ＝１２８/９,w e o b t a i n a p a r t i t i o n S ᶄa n d T ᶄs u c h t h a t e v e r y v e r t e x i n S ᶄh a sa t l e a s t t n e i g h b o r s i n T ᶄ,d (G [S ᶄ])ȡ８s /３,δ(G [T ᶄ])ȡt a n d T ᶄc a nb e o b t a i n e d f r o m T ᵡb y s u c c e s s i v e l y a d d i n g v e r t i c e sh a v i n g a tl e a s t t n e i g h b o r si nt h es u p e r s e to f T ᵡa l r e a d y c o n s t r u c t e d ．T h u s G [T ᶄ]i s t Ｇc o n n e c t e d s i n c e G T ᵡ[]i s ．T h e nb y T h e o r e m２．２,G S ᶄ[]h a s a n s Ｇc o n n e c t e d s u b g r a p h H ．A s e a c hv e r t e xo f S ᶄ\V (H )h a s a t l e a s t t n e i g h b o r s i n T ᶄ,s o G －V (H )i s a l s o t Ｇc o n n e c t e d ．T h e r e f o r e ,S ＝V (H ),T ＝V (G )\S i s a p a r t i t i o na s r e qu i r e d ．３㊀R e m a r kR e c a l l t h a t f o r e a c h p a i r s ,t o f p o s i t i v e i n t e g e r ,g s ,t ()d e n o t e s a n i n t e g e r s s u c h t h a t t h e v e r t e x s e t o f e a c h g s ,t ()Ｇc o n n e c t e d g r a p hc a nb e p a r t i t i o n e d i n t ot w os e t s S a n d T w h i c h i n d u c es u b g r a p h so f c o n n e c t i v i t y a t l e a s t s a n d t ,r e s p e c t i v e l y ．W em a y c o n s i d e r g s ,t ()f o r K r Ｇf r e e g r a p h s s i m i l a r l y ．W e f i n d t h a t e v e r y K r Ｇf r e e g r a p h w i t ha v e r a g ed e g r e ea t l e a s t ４r －１()k /r －２c o n t a i n sa k Ｇc o n n e c t e d s u b g r a p h ．S o l e t G b e a K r Ｇf r e e g r a ph ,i f δ(G )ȡ４r －１()/r s ＋t ()－２,t h e n V (G )c a nb e p a r t i t i o n e d i n t o t w on o n e m p t y s e t s S a n d T s u c ht h a t G [S ],G [T ]a r e s Ｇc o n n e c t e d a n d t Ｇc o n n e c t e d ,r e s p e c t i v e l y ．I n c r e a s e t h em i n i m u md e g r e e o f G u n t i l δ(G )ȡ４r －１()/r s ＋t ()＋t ,t h e n t h e r e i sn o s u b g r a p ho f G [S ]h a s a v e r a g ed e g r e em o r e t h a n ３２(r －１)２s /r ２．F i n a l l y ,w ed e d u c e t h a tw h e n g s ,t ()ȡ２１３ r －１()３s t /r ３,T h e o r e m１．２a l s oh o l d s f o r K r Ｇf r e e g r a p h s ．[R e f e r e n c e s][１]㊀A r i k a t i S ,P a n d uR a n g a nC ．L i n e a r a l g o r i t h mf o ro p t i m a l p a t h p r o b l e mo n i n t e r v a l g r a ph s [J ]．I n f o r m．P r o c e s s ．L e t t ．,１９９０,３５:１４９－１５３．[２]㊀C h a n g GJ ,H o uJ ,R o u s s e lN．O nt h et o t a lc h o o s a b i l i t y o f p l a n a r g r a p h sa n do fs p a r s e g r a ph s [J ]．I n f o r m．P r o c e s s ．L e t t ．,２０１０,１１０:８４９－８５３．[３]㊀C h l e b i k o v áJ ．A p p r o x i m a t i n g t h em a x i m a l l y b a l a n c e dc o n n e c t e d p a r t i t i o n p r o b l e mi n g r a ph s [J ]．I n f o r m．P r o c e s s ．L e t t ．,１９９６,６０:２２５－３３０．[４]㊀D i e s t e lR．G r a p hT h e o r y [M ]．B e r l i n :S p r i n ge r ,１９９７．[５]㊀H a j n a l P ．P a r t i t i o nofg r a ph swi t hc o n d i t i o no nt h ec o n n e c t i v i t y a n d m i n i m u m d e gr e e [J ]．C o m b i n a t o r i c a ,１９８３,３:９５－９９．[６]㊀K üh nD ,O s t h u sD．P a r t i t i o n s o f g r a p h sw i t hh i g h m i n i m u md e g r e eo r c o n n e c t i v i t y [J ]．J ．C o m b i n a t o r i a lT h e o r yS e r ．B ,２００３,８８:２９－４３．[７]㊀L iR ,B a o g a n g X．O na g r a ph p a r t i t i o n r e s u l t o fK üh na n dO s t h u s [J ]．A r sC o m b i n ．,２０１２,１０３:４９１－４９５．[８]㊀L u oX．T h e ４－c h o o s a b i l i t y o f t o r o i d a l g r a p h sw i t h o u t i n t e r s e c t i n g t r i a n gl e s [J ]．I n f o r m．P r o c e s s ．L e t t ．,２００７,１０２:８５－９１．[９]㊀M a d e r W．E x i s t e n zn －f a c hz u s a m m e n h än g e n d e r t e i l g r a p h e n i n g r a p h e n g e n üge n d g r o ße rk a n t e n d i c h t e [J ]．A b h ．M a t h ．S e r e ．H a m b u r g Ut t i v ．,１９７２,３７:８６－９７．[１０]㊀P o l j a kS ,T u z aZ ．M a x i m u mc u t s a n d l a r g eb i p a r t i t e s u b g r a ph s [J ]．D I MA C SS e r i e s i nD i s c r e t eM a t h e m a t i c s a n d T h e o r e t i c a l C o m p u t e r S c i e n c e ,１９９５,２０:１８１－２４４．[１１]㊀S t i e b i t zM．D e c o m p o s i n gg r a p h su n d e r d e g r e e c o n s t r a i n t s [J ]．J ．G r a p hT h e o r y ,１９９６,２３:３２１－３２４．５第５期㊀㊀㊀L IR u i :P a r t i t i o n s o fT r i a n g l e Ｇf r e eG r a p h sw i t hR e s t r i c t i o no nC o n n e c t i v i t y６大㊀学㊀数㊀学㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀㊀第３４卷[１２]㊀T u ránP．E i n eE x t r e m a l a u f g a b e a u s d e rG r a p h e n t h e o r i e[J]．M a t．F i z．L a p o k．,１９４１,４８:４３６－４５２．[１３]㊀T h o m a s s e n C．G r a p hd e c o m p o s i t i o n w i t hc o n s t r a i n t so nt h ec o n n e c t i v i t y a n d m i n i m u m d e g r e e[J]．J．G r a p h T h e o r y,１９８３,７:１６５－１６７．[１４]㊀W a n g Y,L uH,C h e n M．An o t eo n３－c h o o s a b i l i t y o f p l a n a r g r a p h s[J]．I n f o r m．P r o c e s s．L e t t．,２００８,１０５:２０６－２１１．[１５]㊀Y a n JH,C h a n g CJ．T h e p a t h－p a r t i t i o n p r o b l e mi nb l o c k g r a p h s[J]．I n f o r m．P r o c e s s．L e t t．,１９９４,５２:３１７－３２２．[１６]㊀Z h a n g H．O n３－c h o o s a b i l i t y o f p l a n a r g r a p h sw i t hn e i t h e ra d j a c e n t t r i a n g l e sn o r５－,６－a n d９－c y c l e s[J]．I n f o r m．P r o c e s s．L e t t．,２０１０,１１０:１０８４－１０８７．关于连通度的无三圈图的划分李㊀锐(河海大学理学院数学系,南京２１１１００)[摘㊀要]Küh n和O s t h u s证明了对每个正整数l,都存在一个整数k(l)ɤ２１６l２,使得每个k(l)－连通图G的顶点集都可以划分成两个子集S,T满足G[S],G[T]都是l－连通的,且S中的每个点在T中都有l个邻点．本文主要考虑无三圈图的划分问题,主要关注连通度k(l)的上界．通过证明每个平均度至少为８l/３的无三圈图都存在一个lＧ连图子图,我们证明了对无三圈图,k(l)ɤ２１６３－３l２．[关键词]组合问题;划分;连通度;无三圈。

精品高中函数知识点复习总结

精品高中函数知识点复习总结Chapter 2: nsI。

Concept and n of ns1.Mapping1) Mapping: Let A and B be two sets。

If there exists a mapping rule f such that for any element in set A。

there is a unique element in set B that corresponds to it。

then this correspondence (including sets A and B as well as the mappingrule f) is called a mapping from set A to set B。

denoted as f:A→B.2) Image and Preimage: Given a mapping from set A to set B。

the element b in set B that corresponds to element a in set A is called the image of a。

and a is called the preimage of b.Note: (1) Understanding of the n of mapping。

(2) Method to XXX.2.n1) n of n① Original n: XXX variables x and y in a certain process of change。

If for every determined value of x within a certain range。

there is a unique value of y that corresponds to it。

then y is calleda n of x。

and x is called the independent variable.② Modern n: Let A and B be non-empty sets of numbers。

TOP221

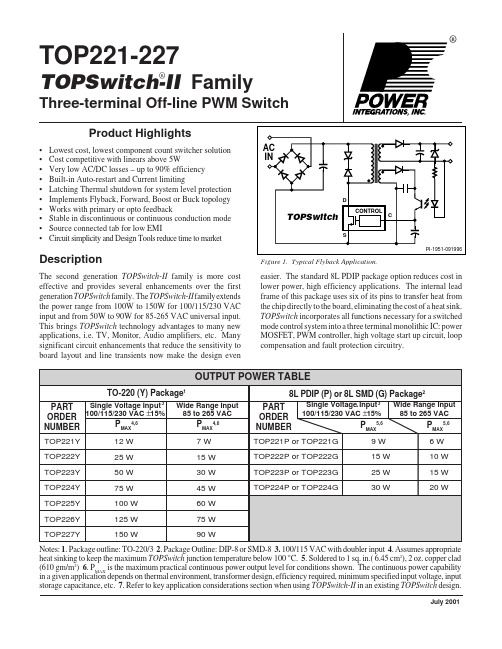
Figure 1. Typical Flyback Application.easier. The standard 8L PDIP package option reduces cost in lower power, high efficiency applications. The internal lead frame of this package uses six of its pins to transfer heat from the chip directly to the board, eliminating the cost of a heat sink.TOPSwitch incorporates all functions necessary for a switched mode control system into a three terminal monolithic IC: power MOSFET, PWM controller, high voltage start up circuit, loop compensation and fault protection circuitry.Product Highlights•Lowest cost, lowest component count switcher solution •Cost competitive with linears above 5W•Very low AC/DC losses – up to 90% efficiency •Built-in Auto-restart and Current limiting•Latching Thermal shutdown for system level protection •Implements Flyback, Forward, Boost or Buck topology •Works with primary or opto feedback•Stable in discontinuous or continuous conduction mode •Source connected tab for low EMI•Circuit simplicity and Design Tools reduce time to marketDescriptionThe second generation TOPSwitch-II family is more cost effective and provides several enhancements over the first generation TOPSwitch family. The TOPSwitch-II family extends the power range from 100W to 150W for 100/115/230 VAC input and from 50W to 90W for 85-265 VAC universal input.This brings TOPSwitch technology advantages to many new applications, i.e. TV, Monitor, Audio amplifiers, etc. Many significant circuit enhancements that reduce the sensitivity to board layout and line transients now make the design evenNotes: 1. Package outline: TO-220/3 2. Package Outline: DIP-8 or SMD-8 3. 100/115 VAC with doubler input 4. Assumes appropriateheat sinking to keep the maximum TOPSwitch junction temperature below 100 °C. 5. Soldered to 1 sq. in.( 6.45 cm 2), 2 oz. copper clad (610 gm/m 2) 6. P MAX is the maximum practical continuous power output level for conditions shown. The continuous power capability in a given application depends on thermal environment, transformer design, efficiency required, minimum specified input voltage, input storage capacitance, etc. 7. Refer to key application considerations section when using TOPSwitch-II in an existing TOPSwitch design.July 2001Figure 2. Functional Block Diagram.Pin Functional DescriptionDRAIN Pin:Output MOSFET drain connection. Provides internal biascurrent during start-up operation via an internal switched high-voltage current source. Internal current sense point.CONTROL Pin:Error amplifier and feedback current input pin for duty cyclecontrol. Internal shunt regulator connection to provide internalbias current during normal operation. It is also used as theconnection point for the supply bypass and auto-restart/compensation capacitor.SOURCE Pin:Y package – Output MOSFET source connection for highvoltage power return. Primary side circuitcommon and reference point.P and G package – Primary side control circuit common andreference point.SOURCE (HV RTN) Pin: (P and G package only)Output MOSFET source connection for high voltage power return.Figure 3. Pin Configuration.D 7/01TOPSwitch-II Family Functional DescriptionTOPSwitch is a self biased and protected linear control current-to-duty cycle converter with an open drain output. High efficiency is achieved through the use of CMOS and integration of the maximum number of functions possible. CMOS process significantly reduces bias currents as compared to bipolar or discrete solutions. Integration eliminates external power resistors used for current sensing and/or supplying initial start-up bias current.During normal operation, the duty cycle of the internal output MOSFET decreases linearly with increasing CONTROL pin current as shown in Figure 4. To implement all the required control, bias, and protection functions, the DRAIN and CONTROL pins each perform several functions as described below. Refer to Figure 2 for a block diagram and to Figure 6 for timing and voltage waveforms of the TOPSwitch integrated circuit.Figure 4. Relationship of Duty Cycle to CONTROL Pin Current.Figure 5. Start-up Waveforms for (a) Normal Operation and (b) Auto-restart.Control Voltage SupplyCONTROL pin voltage VCis the supply or bias voltage for thecontroller and driver circuitry. An external bypass capacitorclosely connected between the CONTROL and SOURCE pinsis required to supply the gate drive current. The total amountof capacitance connected to this pin (CT) also sets the auto-restart timing as well as control loop compensation. VCisregulated in either of two modes of operation. Hystereticregulation is used for initial start-up and overload operation.Shunt regulation is used to separate the duty cycle error signalfrom the control circuit supply current. During start-up,CONTROL pin current is supplied from a high-voltage switchedcurrent source connected internally between the DRAIN andCONTROL pins. The current source provides sufficient currentto supply the control circuitry as well as charge the totalexternal capacitance (CT).The first time VCreaches the upper threshold, the high-voltagecurrent source is turned off and the PWM modulator and outputtransistor are activated, as shown in Figure 5(a). During normaloperation (when the output voltage is regulated) feedbackcontrol current supplies the VCsupply current. The shuntregulator keeps VCat typically 5.7 V by shunting CONTROLpin feedback current exceeding the required DC supply currentthrough the PWM error signal sense resistor RE. The lowdynamic impedance of this pin (ZC) sets the gain of the erroramplifier when used in a primary feedback configuration. Thedynamic impedance of the CONTROL pin together with theexternal resistance and capacitance determines the control loopcompensation of the power system.If the CONTROL pin total external capacitance (CT) shoulddischarge to the lower threshold, the output MOSFET is turnedoff and the control circuit is placed in a low-current standbymode. The high-voltage current source turns on and charges theexternal capacitance again. Charging current is shown with anegative polarity and discharging current is shown with apositive polarity in Figure 6. The hysteretic auto-restartcomparator keeps VCwithin a window of typically 4.7 to 5.7 Vby turning the high-voltage current source on and off as shownin Figure 5(b). The auto-restart circuit has a divide-by-8counter which prevents the output MOSFET from turning onagain until eight discharge-charge cycles have elapsed. Thecounter effectively limits TOPSwitch power dissipation byreducing the auto-restart duty cycle to typically 5%. Auto-restart continues to cycle until output voltage regulation isagain achieved.Bandgap ReferenceAll critical TOPSwitch internal voltages are derived from atemperature-compensated bandgap reference. This referenceis also used to generate a temperature-compensated currentsource which is trimmed to accurately set the oscillator frequencyOscillatorThe internal oscillator linearly charges and discharges theinternal capacitance between two voltage levels to create asawtooth waveform for the pulse width modulator. The oscillatorsets the pulse width modulator/current limit latch at the beginningof each cycle. The nominal frequency of 100 kHz was chosento minimize EMI and maximize efficiency in power supplyapplications. Trimming of the current reference improves thefrequency accuracy.Pulse Width ModulatorThe pulse width modulator implements a voltage-mode controlloop by driving the output MOSFET with a duty cycle inverselyproportional to the current into the CONTROL pin whichgenerates a voltage error signal across RE. The error signalacross REis filtered by an RC network with a typical cornerfrequency of 7 kHz to reduce the effect of switching noise. Thefiltered error signal is compared with the internal oscillatorsawtooth waveform to generate the duty cycle waveform. Asthe control current increases, the duty cycle decreases. A clocksignal from the oscillator sets a latch which turns on the outputMOSFET. The pulse width modulator resets the latch, turningoff the output MOSFET. The maximum duty cycle is set by thesymmetry of the internal oscillator. The modulator has aminimum ON-time to keep the current consumption of theTOPSwitch independent of the error signal. Note that a minimumcurrent must be driven into the CONTROL pin before the dutycycle begins to change.Gate DriverThe gate driver is designed to turn the output MOSFET on at acontrolled rate to minimize common-mode EMI. The gate drivecurrent is trimmed for improved accuracy.Error AmplifierThe shunt regulator can also perform the function of an erroramplifier in primary feedback applications. The shunt regulatorvoltage is accurately derived from the temperature compensatedbandgap reference. The gain of the error amplifier is set by theCONTROL pin dynamic impedance. The CONTROL pinclamps external circuit signals to the VCvoltage level. TheCONTROL pin current in excess of the supply current isseparated by the shunt regulator and flows through REas avoltage error signal.Cycle-By-Cycle Current LimitThe cycle by cycle peak drain current limit circuit uses theoutput MOSFET ON-resistance as a sense resistor. A currentlimit comparator compares the output MOSFET ON-state drain-source voltage, VDS(ON)with a threshold voltage. High draincurrent causes VDS(ON)to exceed the threshold voltage and turnsthe output MOSFET off until the start of the next clock cycle.The current limit comparator threshold voltage is temperature TOPSwitch-II Family Functional Description (cont.)D 7/01compensated to minimize variation of the effective peak current limit due to temperature related changes in output MOSFET R DS(ON).The leading edge blanking circuit inhibits the current limit comparator for a short time after the output MOSFET is turned on. The leading edge blanking time has been set so that current spikes caused by primary-side capacitances and secondary-side rectifier reverse recovery time will not cause premature termination of the switching pulse.The current limit can be lower for a short period after the leading edge blanking time as shown in Figure 12. This is due to dynamic characteristics of the MOSFET. To avoid triggering the current limit in normal operation, the drain current waveform should stay within the envelope shown.Shutdown/Auto-restartTo minimize TOPSwitch power dissipation, the shutdown/auto-restart circuit turns the power supply on and off at an auto-restart duty cycle of typically 5% if an out of regulation condition persists. Loss of regulation interrupts the external current into the CONTROL pin. V C regulation changes from shunt mode to the hysteretic auto-restart mode described above.When the fault condition is removed, the power supply outputbecomes regulated, V C regulation returns to shunt mode, and normal operation of the power supply resumes.Overtemperature ProtectionTemperature protection is provided by a precision analog circuit that turns the output MOSFET off when the junction temperature exceeds the thermal shutdown temperature (typically 135 °C). Activating the power-up reset circuit by removing and restoring input power or momentarily pulling the CONTROL pin below the power-up reset threshold resets the latch and allows TOPSwitch to resume normal power supply operation. V C is regulated in hysteretic mode and a 4.7 V to 5.7 V (typical) sawtooth waveform is present on the CONTROL pin when the power supply is latched off.High-voltage Bias Current SourceThis current source biases TOPSwitch from the DRAIN pin and charges the CONTROL pin external capacitance (C T ) during start-up or hysteretic operation. Hysteretic operation occurs during auto-restart and overtemperature latched shutdown.The current source is switched on and off with an effective duty cycle of approximately 35%. This duty cycle is determined by the ratio of CONTROL pin charge (I C ) and discharge currents (I CD1 and I CD2). This current source is turned off during normal operation when the output MOSFET is switching.Figure 6. Typical Waveforms for (1) Normal Operation, (2) Auto-restart, and (3) Power Down Reset.Figure 7. Schematic Diagram of a 4 W TOPSwitch-II Standby Power Supply using an 8 lead PDIP.Application ExamplesFollowing are just two of the many possible TOPSwitchimplementations. Refer to the Data Book and Design Guidefor additional examples.4 W Standby Supply using 8 Lead PDIPFigure 7 shows a 4 W standby supply. This supply is used inappliances where certain standby functions (e.g. real timeclock, remote control port) must be kept active even while themain power supply is turned off.The 5 V secondary is used to supply the standby function andthe 12 V non-isolated output is used to supply power for thePWM controller of the main power supply and other primaryside functions.For this application the input rectifiers and input filter are sizedfor the main supply and are not shown. The input DC rail mayvary from 100 V to 380 V DC which corresponds to the fulluniversal AC input range. The TOP221 is packaged in an 8 pinpower DIP package.The output voltage (5 V) is directly sensed by the Zener diode(VR1) and the optocoupler (U2). The output voltage is determinedby the sum of the Zener voltage and the voltage drop across theLED of the optocoupler (the voltage drop across R1 is negligible).The output transistor of the optocoupler drives the CONTROLpin of the TOP221. C5 bypasses the CONTROL pin and providescontrol loop compensation and sets the auto-restart frequency.The transformer’s leakage inductance voltage spikes are snubbedby R3 and C1 through diode D1. The bias winding is rectifiedand filtered by D3 and C4 providing a non-isolated 12 V outputwhich is also used to bias the collector of the optocoupler’soutput transistor. The isolated 5 V output winding is rectified byD2 and filtered by C2, L1 and C3.D 7/0120 W Universal Supply using 8 Lead PDIPFigure 8 shows a 12 V, 20 W secondary regulated flyback power supply using the TOP224P in an eight lead PDIP package and operating from universal 85 to 265 VAC input voltage. This example demonstrates the advantage of the higher power 8 pin leadframe used with the TOPSwitch-II family. This low cost package transfers heat directly to the board through six source pins, eliminating the heatsink and the associated cost. Efficiency is typically 80% at low line input. Output voltage is directly sensed by optocoupler U2 and Zener diode VR2. The output voltage is determined by the Zener diode (VR2) voltage and the voltage drops across the optocoupler (U2) LED and resistor R1.Other output voltages are possible by adjusting the transformer turns ratio and value of Zener diode VR2.AC power is rectified and filtered by BR1 and C1 to create the high voltage DC bus applied to the primary winding of T1. The other side of the transformer primary is driven by the integrated TOPSwitch-II high-voltage MOSFET. D1 and VR1 clampleading-edge voltage spikes caused by transformer leakage inductance. The power secondary winding is rectified and filtered by D2, C2, L1, and C3 to create the 12 V output voltage.R2 and VR2 provide a slight pre-load on the 12 V output to improve load regulation at light loads. The bias winding is rectified and filtered by D3 and C4 to create a TOPSwitch bias voltage. L2 and Y1-safety capacitor C7 attenuate common mode emission currents caused by high voltage switching waveforms on the DRAIN side of the primary winding and the primary to secondary capacitance. Leakage inductance of L2with C1 and C6 attenuates differential-mode emission currents caused by the fundamental and harmonics of the trapezoidal or triangular primary current waveform. C5 filters internal MOSFET gate drive charge current spikes on the CONTROL pin, determines the auto-restart frequency, and together with R1 and R3, compensates the control loop.Figure 8. Schematic Diagram of a 20 W Universal Input TOPSwitch-II Power Supply using an 8 lead PDIP.Key Application ConsiderationsGeneral Guidelines• Keep the SOURCE pin length very short. Use a Kelvinconnection to the SOURCE pin for the CONTROL pinbypass capacitor. Use single point grounding techniques atthe SOURCE pin as shown in Figure 9.• Minimize peak voltage and ringing on the DRAIN voltageat turn-off. Use a Zener or TVS Zener diode to clamp thedrain voltage below the breakdown voltage rating ofTOPSwitch under all conditions, including start-up andoverload. The maximum recommended clamp Zenervoltage for the TOP2XX series is 200 V and thecorresponding maximum reflected output voltage on theprimary is 135 V. Please see Step 4: AN-16 in the 1996-97Data Book and Design Guide or on our Web site.• The transformer should be designed such that the rate ofchange of drain current due to transformer saturation iswithin the absolute maximum specification (∆IDin 100 nsbefore turn off as shown in Figure 13). As a guideline, formost common transformer cores, this can be achieved bymaintaining the Peak Flux Density (at maximum ILIMITcurrent) below 4200 Gauss (420 mT). The transformerspreadsheets Rev. 2.1 (or later) for continuous and Rev.1.0(or later) for discontinuous conduction mode provide thenecessary information.• Do not plug TOPSwitch into a “hot” IC socket during test.External CONTROL pin capacitance may be charged toexcessive voltage and cause TOPSwitch damage.• While performing TOPSwitch device tests, do not exceedmaximum CONTROL pin voltage of 9 V or maximumCONTROL pin current of 100 mA.• Under some conditions, externally provided bias or supplycurrent driven into the CONTROL pin can hold theTOPSwitch in one of the 8 auto-restart cycles indefinitelyand prevent starting. To avoid this problem when doingbench evaluations, it is recommended that the VCpowersupply be turned on before the DRAIN voltage is applied.TOPSwitch can also be reset by shorting the CONTROLpin to the SOURCE pin momentarily.• CONTROL pin currents during auto-restart operation aremuch lower at low input voltages (< 36 V) which increasesthe auto-restart cycle time (see the ICvs. DRAIN VoltageCharacteristic curve).• Short interruptions of AC power may cause TOPSwitch toenter the 8-count auto-restart cycle before starting again.This is because the input energy storage capacitors are notcompletely discharged and the CONTROL pin capacitancehas not discharged below the internal power-up resetvoltage.• In some cases, minimum loading may be necessary to keepa lightly loaded or unloaded output voltage within thedesired range due to the minimum ON-time.Replacing TOPSwitch with TOPSwitch-IIThere is no external latching shutdown function inTOPSwitch-II. Otherwise, the functionality of theTOPSwitch-II devices is same as that of the TOPSwitch family.However, before considering TOPSwitch-II as a 'drop in'replacement in an existing TOPSwitch design, the designshould be verified as described below.The new TOPSwitch-II family offers more power capabilitythan the original TOPSwitch family for the same MOSFETRDS(ON). Therefore, the original TOPSwitch design must bereviewed to make sure that the selected TOPSwitch-IIreplacement device and other primary components are not overstressed under abnormal conditions.The following verification steps are recommended:• Check the transformer design to make sure that it meets the∆IDspecification as outlined in the General Guidelinessection above.• Thermal: Higher power capability of the TOPSwitch-IIwould in many instances allow use of a smaller MOSFETdevice (higher RDS(ON)) for reduced cost. This may affectTOPSwitch power dissipation and power supply efficiency.Therefore thermal performance of the power supply mustbe verified with the selected TOPSwitch-II device.• Clamp Voltage: Reflected and Clamp voltages should beverified not to exceed recommended maximums for theTOP2XX Series: 135 V Reflected/200 V Clamp. Pleasesee Step 4: AN-16 in the Data Book and Design Guide andreadme.txt file attached to the transformer designspreadsheets.• Agency Approval: Migrating to TOPSwitch-II may requireagency re-approval.D 7/01Figure 9. Recommended TOPSwitch Layout.Design ToolsThe following tools available from Power Integrations greatly simplify TOPSwitch based power supply design.• Data Book and Design Guide includes extensive application information• Excel Spreadsheets for Transformer Design - Use of this tool is strongly recommended for all TOPSwitch designs.• Reference design boards – Production viable designs that are assembled and tested.All data sheets, application literature and up-to-date versions of the Transformer Design Spreadsheets can be downloaded from our Web site at . A diskette of the Transformer Design Spreadsheets may also be obtained by sending in the completed form provided at the end of this data sheet.D 7/01D 7/01NOTES:A.For specifications with negative values, a negative temperature coefficient corresponds to an increase in magnitude with increasing temperature, and a positive temperature coefficient corresponds to a decrease in magnitude with increasing temperature.B.The breakdown voltage and leakage current measurements can be accomplished as shown in Figure 15 by using the following sequence:i. The curve tracer should initially be set at 0 V. The base output should be adjusted through a voltage sequence of 0 V, 6.5 V, 4.3 V, and 6.5 V, as shown. The base current from the curve tracer should not exceed 100 mA. This CONTROL pin sequence interrupts the Auto-restart sequence and locks the TOPSwitch internal MOSFET in the OFF State.ii. The breakdown and the leakage measurements can now be taken with the curve tracer. The maximum voltage from the curve tracer must be limited to 700 V under all conditions.C.It is possible to start up and operate TOPSwitch at DRAIN voltages well below 36 V. However, the CONTROL pin charging current is reduced, which affects start-up time, auto-restart frequency, and auto-restart duty cycle. Refer to the characteristic graph on CONTROL pin charge current (I C ) vs. DRAIN voltage for low voltage operation characteristics.Figure 11. TOPSwitch CONTROL Pin I-V Characteristic. Figure 10. TOPSwitch Duty Cycle Measurement.Figure 12. Self-protection Current Limit Envelope.Figure 13. Example of ∆IDon Drain Current Waveform withSaturated Transformer.012683Time (µs)DRAINCurrent(normalized)PI-222-331457120100804020600246810CONTROL Pin Voltage (V)CONTROLPinCurrent(mA)D 7/01Figure 14. TOPSwitch General Test Circuit.Figure 15. Breakdown Voltage and Leakage Current Measurement Test Circuit.The following precautions should be followed when testingTOPSwitch by itself outside of a power supply. The schematicshown in Figure 14 is suggested for laboratory testing ofTOPSwitch.When the DRAIN supply is turned on, the part will be in theAuto-restart mode. The CONTROL pin voltage will beoscillating at a low frequency from 4.7 to 5.7 V and the DRAINis turned on every eighth cycle of the CONTROL pin oscillation.If the CONTROL pin power supply is turned on while in thisTypical Performance CharacteristicsAuto-restart mode, there is only a 12.5% chance that the controlpin oscillation will be in the correct state (DRAIN active state)so that the continuous DRAIN voltage waveform may beobserved. It is recommended that the VCpower supply beturned on first and the DRAIN power supply second if continuousdrain voltage waveforms are to be observed. The 12.5% chanceof being in the correct state is due to the 8:1 counter. Temporarilyshorting the CONTROL pin to the SOURCE pin will resetTOPSwitch, which then will come up in the correct state.21.21.6020*********DRAIN Voltage (V)CONTROLPinChargingCurrent(mA)I C vs. DRAIN VOLTAGEPI-1145-131940.40.81.11.00.9-50-250255075100125150Junction Temperature (°C)BreakdownVoltage(V)(Normalizedto25°C)BREAKDOWN vs. TEMPERATUREPI-176B-513911.21.00.80.60.40.2-50-250255075100125150Junction Temperature (°C)CURRENT LIMIT vs. TEMPERATUREPI-1125-331CurrentLimit(Normalizedto25°C)1.21.00.80.60.40.2-50-250255075100125150Junction Temperature (°C)FREQUENCY vs. TEMPERATUREPI-1123A-331OutputFrequency(Normalizedto25°C)D 7/01Typical Performance Characteristics (cont.)3246810DRAIN Voltage (V)D R A I N C u r r e n t (A )OUTPUT CHARACTERISTICSP I -1940-03300112100010400200600DRAIN Voltage (V)D R A I N C a p a c i t a n c e (p F )C OSS vs. DRAIN VOLTAGE100P I -1941-033001500300400100200200400600DRAIN Voltage (V)P o w e r (m W )DRAIN CAPACITANCE POWERP I -1942-0330017/01Notes-1) Updated package references.2) Corrected Spelling.3) Corrected Storage Temperature θJCand updated nomenclature in parameter table.4) Added G package references to Self-Protection Current Limit parameter.5) Corrected font sizes in figures.Date12/977/01 RevisionCDKOREAPower IntegrationsInternational Holdings, Inc.Rm# 402, Handuk Building649-4 Yeoksam-Dong,Kangnam-Gu,Seoul, KoreaPhone:+82-2-568-7520Fax:+82-2-568-7474e-mail: koreasales@WORLD HEADQUARTERSAMERICASPower Integrations, Inc.5245 Hellyer AvenueSan Jose, CA 95138 USAMain:+1 408-414-9200Customer Service:Phone:+1 408-414-9665Fax:+1 408-414-9765e-mail: usasales@For the latest updates, visit our Web site:Power Integrations reserves the right to make changes to its products at any time to improve reliability or manufacturability. Power Integrations does not assume any liability arising from the use of any device or circuit described herein, nor does it convey any license under its patent rights or the rights of others.The PI Logo, TOPSwitch, TinySwitch and EcoSmart are registered trademarks of Power Integrations, Inc.©Copyright 2001, Power Integrations, Inc.JAPANPower Integrations, K.K.Keihin-Tatemono 1st Bldg.12-20 Shin-Yokohama 2-ChomeKohoku-ku, Yokohama-shiKanagawa 222-0033, JapanPhone:+81-45-471-1021Fax:+81-45-471-3717e-mail: japansales@TAIWANPower IntegrationsInternational Holdings, Inc.17F-3, No. 510Chung Hsiao E. Rd.,Sec. 5,Taipei, Taiwan 110, R.O.C.Phone:+886-2-2727-1221Fax:+886-2-2727-1223e-mail: taiwansales@EUROPE & AFRICAPower Integrations (Europe) Ltd.Centennial CourtEasthampstead RoadBracknellBerkshire, RG12 1YQUnited KingdomPhone:+44-1344-462-300Fax:+44-1344-311-732e-mail: eurosales@CHINAPower IntegrationsInternational Holdings, Inc.Rm# 1705, Bao Hua Bldg.1016 Hua Qiang Bei LuShenzhen, Guangdong 518031ChinaPhone:+86-755-367-5143Fax:+86-755-377-9610e-mail: chinasales@INDIA (Technical Support)Innovatech#1, 8th Main RoadVasanthnagarBangalore, India 560052Phone:+91-80-226-6023Fax:+91-80-228-9727e-mail: indiasales@APPLICATIONS HOTLINEWorld Wide +1-408-414-9660APPLICATIONS FAXWorld Wide +1-408-414-9760。

Interpolation Using Prelookup翻译

Interpolation Using Prelookup :: Blocks (Simulink®) jar:file:///D:/Program%20Files/matlab/help/toolbox/simulink/help.jar!/slr...®SimulinkProvide feedback about this page Interpolation Using PrelookupUse output of Prelookup block to accelerate approximation of N-dimensional functionLibraryLookup TablesDescriptionThe Interpolation Using Prelookup block is intended for use with the Prelookup block. The Prelookup block calculates the index and interval fraction计算索引值和间隔部分 that specifies how its input value relates to the breakpoint data set. You feed将..供给 the resulting index and fraction values into an Interpolation Using Prelookup block to interpolate内插 an n-dimensional table. This combination of blocks performs the equivalent operation模块组合与…有等效的作用 that a single instance of the Lookup Table (n-D) block performs. However, the Prelookup and Interpolation Using Prelookup blocks offer greater flexibility更加灵活that can provide more efficient simulation and code generation更加高效的仿真和代码生成.To use this block, you must define a set of output values as the Table data parameter定义输出值作为列表数据参数. In normal use, these table values correspond to the breakpoint data sets specified in Prelookup blocks列表值与在Prelookup中指定的断点数据相对应. The Interpolation Using Prelookup block generates its output by looking up or estimating table values 通过查表或估算产生输出值 based on the index and interval fraction values (denoted on the block as k a nd f, respectively) fed into the block by each Prelookup block:If the inputs match等于 the values of indices specified in breakpoint data sets, the InterpolationUsing Prelookup block outputs the table value at the intersection of the row, column, 输出行列的交点表值and higher dimension breakpoints.If the inputs do not match不等于 the values of indices specified in breakpoint data sets, theInterpolation Using Prelookup block generates output by interpolating appropriate table values插值适当的表值. If the inputs are beyond the range of breakpoint data sets, the Interpolation UsingPrelookup block can extrapolate its output value外推输出值.The Interpolation Using Prelookup block can perform interpolation on a portion of its table在表的部分进行插值. The Number of sub-table selection dimensions子表选择维数parameter lets you specify that interpolation occur only on a subset of its Table data 仅在表数据的一个子集parameter. For example, if your 3-D table data constitutes a stack of 2-D tables to be interpolated, set the Number of sub-table selection dimensions parameter to 1. The block displays an input port (labeled as sel) used to select and interpolate the 2-D tables.Data Type SupportThe Interpolation Using Prelookup block accepts real signals of any numeric data type任何数字数据类型 supported by Simulink software, except Boolean. The Interpolation Using Prelookup block supports fixed-point data types for signals, table data, and intermediate results.Interpolation Using Prelookup :: Blocks (Simulink®) jar:file:///D:/Program%20Files/matlab/help/toolbox/simulink/help.jar!/slr... For a discussion on the data types supported by Simulink software, see Data Types Supported by Simulink in the Simulink documentation.Parameters and Dialog BoxThe Main pane of the Interpolation Using Prelookup block dialog box appears as follows:Number of table dimensionsThe number of dimensions that the Table data parameter must have表数据必须具有的维数. This determines the number of independent variables for the table and hence the number of inputs to the block表独立变量的数量和输入量的数. Enter an integer between 1 a nd 30 i nto this field.Table dataThe table of output values输出值表. During simulation, the matrix size must match the dimensions defined by the Number of table dimensions parameter. Note that during block diagram editing, you can enter an empty matrix空矩阵 (specified as []) or an undefined workspace variable一个未定义的工作区变量 as the Table data parameter. Use this behavior to postpone specifying acorrectly-dimensioned matrix for the Table data parameter and continue editing the block diagram.For information about how to construct multidimensional arrays如何构建多维数组 in MATLAB software, see M ultidimensional Arrays in the MATLAB online documentation.Click the Edit button to open the Lookup Table Editor (see Lookup Table Editor in theSimulink documentation).Interpolation method插值法None – Flat o r Linear. See Interpolation Methods in the Simulink documentation for moreinformation.Extrapolation method外推法None – Clip o r Linear. See Extrapolation Methods in the Simulink documentation for moreinformation. The Extrapolation method parameter is visible外推方法的参数是可见的only if youselect Linear as the Interpolation method parameter.Note The Interpolation Using Prelookup block does not support Linearextrapolation if its input or output signals specify integer or fixed-point data types整数或定点数据类型.Action for out of range inputSpecifies whether to produce a warning or error message if the input is out of range指定是否将产生一个警告或错误信息，如果输入超出范围. The options are:None — the default, no warning or error messageWarning — display a warning message in the MATLAB Command Window andcontinue the simulationError — halt停止 the simulation and display an error message in the SimulationDiagnostics ViewerCheck index in generated code (Real-Time Workshop license required)Specifies whether Real-Time Workshop software generates code that checks the validity of the indexvalues fed to this block.Valid index input may reach last indexSpecifies how the index and interval fraction inputs to the block (labeled respectively as k a nd f o n theblock) access the last elements of the n-dimensional table specified by the Table data parameter. Ifenabled, the block returns the value of the last element in a particular dimension of its table when kindexes the last table element in the corresponding dimension and f i s 0. If disabled, the block returnsthe value of the last element in a particular dimension of its table when k i ndexes the next-to-last tableelement in the corresponding dimension and f i s 1. Note that index values are zero-based.This parameter is visible only if the Interpolation method specifies Linear a nd theExtrapolation method is None - Clip.Number of sub-table selection dimensionsSpecifies the number of dimensions of the subtable used to compute this block's output. Specify0 (the default) to interpolate the entire table, which disables subtable selection禁用子表选择.Sample timeSpecify the time interval 指定时间间隔between samples. To inherit the sample time, set this parameter to-1. See How to Specify the Sample Time in the Simulink documentation for more information. The Signal Attributes pane of the Interpolation Using Prelookup block dialog box appears as follows:Output minimum输出的最小值Specify the minimum value that the block should output. The default value, [], is equivalent to -Inf.Simulink software uses this value to perform:Parameter range checking (see Checking Parameter Values)Simulation range checking (see Checking Signal Ranges)Automatic scaling自动缩放 of fixed-point data typesOutput maximum输出的最大值Specify the maximum value that the block should output. The default value, [], is equivalent to Inf.Simulink software uses this value to perform:Parameter range checking (see Checking Parameter Values)Simulation range checking (see Checking Signal Ranges)Automatic scaling of fixed-point data typesOutput data type输出数据类型Specify the output data type. You can set it to:A rule that inherits a data type继承数据类型规则, for example, Inherit: Inherit viaback propagation反向传播法The name of a built-in data type内置数据类型, for example, singleThe name of a data type object, for example, a Simulink.NumericType o bjectAn expression that evaluates to a data type, for example, fixdt(1,16,0)Click the Show data type assistant button to display the Data Type Assistant, which helps you set the Output data type parameter.See Specifying Block Output Data Types for more information.Lock scaling against changes by the autoscaling toolSelect to lock scaling of data选择锁定缩放数据.Integer rounding mode数据舍入模式Select the rounding mode for fixed-point operations. For more information, see R ounding in theSimulink Fixed Point User's Guide.Block parameters such as Table data are always rounded to the nearest representable value最接近的可表示值. To control the rounding of a block parameter, enter an expression using a MATLABrounding function into the mask field.Saturate on integer overflow整数溢出饱和Select to have overflows saturate溢出饱和. Otherwise, overflows wrap.When you select this check box, saturation applies to every internal operation on the block, not just the output or result. In general, the code generation process can detect when overflow is not possible, in which case, no saturation code is generated.The Table Attributes pane of the Interpolation Using Prelookup block dialog box appears as follows:Table minimum表最小值Specify the minimum value for table data. The default value, [], is equivalent to -Inf.Table maximum表最大值Specify the maximum value for table data. The default value, [], is equivalent to Inf.Table data typeSpecify the table data type. You can set it to:A rule that inherits a data type, for example, Inherit: Same as outputThe name of a built-in data type, for example, singleThe name of a data type object, for example, a Simulink.NumericType o bjectAn expression that evaluates to a data type, for example, fixdt(1,16,0)Tip Specify a table data type different from the output data type for these cases:Interpolation Using Prelookup :: Blocks (Simulink®) jar:file:///D:/Program%20Files/matlab/help/toolbox/simulink/help.jar!/slr...Lower memory requirement for storing table data that uses a smaller type than theoutput signalSharing of prescaled table data between two Interpolation Using Prelookup blockswith different output data typesSharing of custom storage table data in Real-Time Workshop generated code forblocks with different output data typesThe Intermediate Attributes中间值特性pane of the Interpolation Using Prelookup block dialog box appears as follows:Intermediate results data typeSpecify the intermediate results data type. You can set it to:A rule that inherits a data type, for example, Inherit: Same as outputThe name of a built-in data type, for example, singleThe name of a data type object, for example, a Simulink.NumericType o bjectAn expression that evaluates to a data type, for example, fixdt(1,16,0)Interpolation Using Prelookup :: Blocks (Simulink®) jar:file:///D:/Program%20Files/matlab/help/toolbox/simulink/help.jar!/slr...Click the Show data type assistant button to display the Data Type Assistant,which helps you set the Intermediate results data type parameter.Tip Use this parameter to specify higher precision指明更高的精度for internalcomputations than for table data or output data.CharacteristicsSee AlsoPrelookupProvide feedback about this pageIntegrator Interval Test © 1984-2009 The MathWorks, Inc. •Terms of Use •Patents •Trademarks •Acknowledgments。

图论总结(超强大)解读

UHFLI 600 MHz锁相放大器 - 产品说明书

UHFLI600MHz Lock-in Amplifier Zurich Instruments2Input Channels,2Signal Outputs Ultra-low time constantProduct LeafletRelease Date:January2019Key FeaturesDC to600MHz,1.8GSa/s,12bit4nV/√Hz input noise,100dB dynamic reserve, 30ns minimum time constant8demodulators,up to8oscillatorsLabOne®Toolset:Scope,Imaging Module, FFTSpectrum Analyzer,Parametric SweeperAPIs for LabVIEW®,.NET,MATLAB®,C and PythonSummaryThe Zurich Instruments UHFLI is a ultra-high-frequency dual channel lock-in amplifier that sets the industry standard in terms of frequency range,demodulation bandwidth,integrated tool set and control software.To meet the requirements of the most demanding applica-tions the basic instrument functionality can be extended with the following options:UHF-BOX Boxcar AveragerUHF-AWG Arbitrary Waveform GeneratorUHF-DIG DigitizerUHF-MF Multi-frequencyUHF-PID Quad PID/PLL ControllerUHF-MOD AM/FM ModulationUHF-CNT Pulse CounterThese options are upgradeable in the field.For applica-tions that require a high-accuracy frequency reference the UHF-RUB Rubidium atomic clock is available as a hardware option.DescriptionSignal Inputs and OutputsThe two12bit signal inputs exhibit a minimum noise of 4nV/√Hz.The1.8GSa/s sampling rate ensures suffi-cient aliasing suppression and high SNR.Linear combi-nations of multiple sinusoids can be generated at14bit resolution in multiple ranges up to±1.5V.The UHF-MF Multi-frequency option increases the number of oscilla-tors from2to8.Arbitrary waveforms can be generated with the UHF-AWG option installed.Demodulators and FiltersThe filters of all8dual-phase demodulators can be indi-vidually configured.The digital filters offer a much higher dynamic reserve,zero drift,precise phase shifts,and perfect orthogonality in contrast to their analog coun-terparts.All demodulated signals can be output with a bandwidth of up to5MHz on4auxiliary outputs with 16bit resolution.LabOne is Instrument ControlThe UHFLI includes the LabOne control software.Thanks to the latest web server technology,the user interface can be easily accessed from any browser.With LabOne the computer is the cockpit for instrument control,data capture,analysis and storage where every setting is no more than2clicks away.The functionality includes an Oscilloscope,a Spectrum Analyzer,an Imaging Module, a Plotter and a Parametric Sweeper for quick and easy measurement automation and much more.Choice of APIsFor convenient integration into existing control environ-ments,programming interfaces for LabVIEW,.NET,MAT-LAB,C and Python are provided.LabOne UserInterfaceLock-in AmplifierEvery demodulator has a graphical representation in the form of a block-diagram for intuitive instrument control.In addition,the overview tab allows the control of all de-modulators,signal inputs and signal outputs from a sin-glepanel.Oscilloscope with FFTTime and frequency domain analysis of signal inputs and trigger signals with the following key features: Full input A/D resolution:12bit,1.8GSa/sCursor math:Location,Area,Tracking,Wave,Peak,Histogram2independent channels,128MSa segmented waveform memory with UHF-DIGoption Parametric SweeperThe Parametric Sweeper enables the user to automate measurements by scanning instrument parameters over a defined range with a freely adjustable number of scan steps,either linearly or logarithmically.The recording of frequency dependence as well as the variation of bias voltages or test signal amplitudes can be easily auto-mated.A variety of application modes help the user to measure with optimum settings and get the most accu-rate results in a minimum of measurement time without manual tweaking. Sweep parameters:frequency,phase shift,output amplitude,signal offset,etc.Frequency response analyzer (Bode plots) Application modes:FRA,Noise,3-Omega,etc.Normalization,auto bandwidth,averaging and standard deviationSpectrumFFT Spectrum AnalyzerThe Spectrum Analyzer takes any of the demodulators’signals or frequency as an input and applies a fast Fourier transform.The main features are: Modes:FFT(X+iY),FFT(R),FFT(ϴ),FFT(f)and FFT((dϴ/dt)/2π)Auto bandwidth,auto span,filter compensation 4different FFT window functionsAmplitude,spectral density and powerspectrum Plotter & Data AcquisitionThe Plotter and Data Acquisition module display multi-ple measurement data in the time domain.The Plotter displays the data continuously and the Data Acquisition module captures and displays individual shots based on numerous internal and external trigger conditions. Multi-trace support with axis grouping for flexible axis scalingPolar and Cartesian data formatCursor math:Location,Area,Tracking,Wave,Peak,Histogram ImagingImaging ModuleThe imaging module converts any of the measurement signals into images and supports: A clear definition of a ”line”based on a line trigger and a user-defined durationResampling to a defined number of pixels with a suitable interpolation and/or averagingStore images in different file formatsZurich Instruments –Product Leaflet:UHFLI 600MHz Lock-in AmplifierUpgrade OptionsUHF-MFMulti-frequency8oscillators instead of 2 Arbitrary choice of frequencyOutput adder for 8sinusoidal signalsThe UHF-MF option adds another 6internal oscillators to the instrument and allows a free choice of frequency for each of the 8demodulators.This extends the capability of the Instrument by offering a wide range of additional configurations for measurement and signal generation.UHF-DIGDigitizerDual-trace oscilloscope with FFT 128MSa memory per channel Segmented memoryFast continuous data streamingThe combination of Lock-in amplifier,AWG and Digitizer in one instrument enables a wide range of new mea-surement opportunities utilizing continuous streaming,sophisticated cross-domain triggering and a segmented 128MSa memory for each of the 2Scope channels.UHF-PID Quad PID/PLLController4independent PID controllers300kHz maximum loop filter bandwidth LabOne PID Advisor and Auto-tune ±1024πphase-unwrapThe 4PID controllers are seamlessly integrated into the lock-in and can take all input and measurement signals as inputs and provide feedback via signal amplitudes,phase shifts,signal offsets,auxiliary outputs and more.The LabOne PID Advisor and Auto-tune features support you to achieve locking quickly and with high performance.UHF-MOD AM/FMModulationAM and FM modulation/demodulation Single sideband operationHigher harmonics of carriers and higher order sidebandsMeasure at up to 2phase coherent linear combinations of 2oscillator frequencies.The filter for each frequency component can be individually configured.-0.75-0.70-0.65-0.60-0.55-0.50-0.45-0.40-0.35-0.30-0.25-0.20-0.15-0.10-0.05-0.00-4-3-2-1-01234-0.6-0.4-0.2-0.00.20.4Wave Channel 1Wave Channel 2UHF-BOX Boxcar Averager2Boxcar unitsBaseline suppression2Periodic Waveform Analyzers (PWA)Zero acquisition dead timeThe UHF-BOX is the first fully digitalboxcar averager en-abling a performance step-up analyzing non-sinusoidal signals with small duty cycles.It operates as a high speed digitizer synchronized to an oscillator and therefore cap-tures every sample without any dead time while reject-ing all non-periodic signal components.With the Periodic Waveform Analyzer (PWA)you can visualize the averaged signal over a single or multiple periods and set the boxcar and the baseline suppression windows.UHF-CNT Pulse Counter4counter modules225MHzmaximum count rate Adjustable discriminator levelBackground subtractionThe UHF-CNT Pulse Counter features analysis of up to 4pulse trains in parallel,enabling event based mea-surements,e.g.photon detection using photo-multiplier tubes.There are five distinct modes of operation and each channel offers an adjustable discriminator.Through the cross-domain trigger,branching conditions for the UHF-AWG can be defined based on counter val-ues,enabling ultra-fast feed-forward loops for quantum error correction.Waveform GeneratorDual 600MHz AWG600MHz bandwidth,1.8GSa/s 14-bit vertical resolutionAmplitude modulationThe UHF-AWG option brings state-of-the-art arbitrary waveform generator (AWG)capabilities to the UHFLI,the world’s fastest and most advanced lock-in amplifier.The result is a very powerful combination of complex signal generation and analysis tools conveniently combined in LabOne ®,the intuitive and platform-independent instru-ment control software.The figure below shows a dual-channel AWG signal captured with the UHF-DIG Digitizer using the internal cross-domain trigger.Zurich Instruments –Product Leaflet:UHFLI 600MHz Lock-in AmplifierSpecificationZurichInstrumentsTechnoparkstrasse 1CH-8005 Zurich Switzerland ***************+41 44 515 04 10Disclaimer: The content of this document is provided by Zurich Instruments ‘as is’.Zurich Instruments makes no warranties with respect to the accuracy or completeness of the content of this document and reserves the right to make changes to the specification at any time without notice. All trademarks are the property of their respective owners.。

The number of maximum independent sets in graphs

运筹学英汉词汇ABC

数学专业英语2-10翻译

偏最小二乘法(pls)简介(Partial least squares (PLS) Introduction)

数学英语如何用英语表达数学术语

数学英语词汇(初等数学)

IQview使用说明

某咨询国际的中英文双语作品国际职位评估系统

图论总结(超强大)解读

CCA

Datalogic 激光扫描仪和光幕用户手册说明书

XOR-based

dynamic programming 动态规划

SADISA包（版本1.2）：物种丰度分布与独立物种假设说明书

小学上册A卷英语自测题(有答案)

关于连通度的无三圈图的划分

精品 高中函数知识点复习总结

TOP221

Interpolation Using Prelookup翻译

图论总结(超强大)解读

UHFLI 600 MHz锁相放大器 - 产品说明书

精品高中函数知识点复习总结