Octave_Bands

合集下载

STC,MTC,OITCandRwExplanation

Calculation of:STC (Sound Transmission Class),R W (European) as per ISO 717-1, MTC (Music/Machinery Transmission Class),OITC (Outdoor-Indoor Transmission Class)Note: This document is an extension/adaptation of a document explaining STC and OITC to be found at /acoustical/STCandOITC.docIt is expanded (+ additional adjustments and more in-depth comments) with the MTC and R W single number rating. The related mathematical MTC approach was explained by Dr. Noral D. Stewart a US acoustic consultant, member of ASTM to the Author Eric Desart, who adjusted/expanded this document for use in the studiotips acoustics group.The additional MTC single number rating was designed by H. Stanley Roller, Architectural Construction Manager, Acoustics, United States Gypsum Company, in order to compensate for the neglect of the STC Class in view of low frequent noise as music and machinery.TL: Transmission Loss · · · ASTM E-90TL values per frequency band in one-third octave bands to be used for the calculation of the single number ratings are obtained as per ASTM E-90 and rounded to the nearest whole dB TL value.This deviate from the European and ISO approach, which mostly use TL values rounded to 1/10 dB. The difference between both approaches can cause differences on the rounded re-sulting single number ratings of 1 dB. Suggestions were made within ASTM meetings to alter the ASTM E-90 approach to an accuracy of 1/10 dB. However most likely the standard used method of rounding TL values to whole dB values will continue to exist.STC: Sound Transmission Class · · · ASTM E413-87 (Re-approved 1994)Classification for Rating Sound InsulationThe Sound Transmission Class (STC) method assigns a single number rating to measured Sound Transmission Loss (TL) data obtained in accordance with ASTM E-90. The method compares a family of numbered contours with one-third octave band TL data covering the one-third octave bands from 125 Hz to 4000 Hz, inclusive. The number of the contour that best fits the data gives the STC rating.All contours are defined across the one-third octave band range 125 Hz to 4000 Hz, inclusive, and are numbered according to their value at the 500 Hz band. The numbered contours are generated from a reference contour, which defines STC 0. The one-third octave band values of a numbered contour are computed by adding the contour number (which must be an inte-ger) to the reference contour values for all bands.For example, the 45 contour has a value of 45 dB in the 500 Hz band, as well as 29 dB (45–16 dB) in the 125 Hz band, 32 dB (45-13 dB) in the 160 Hz band, and so on.To calculate the STC,1.Select a trial contour for comparison with the measured TL data.2.Identify bands with “deficiencies”, where the measured TL data fall below the co n-tour under consideration. The magnitude of the deficiency is the difference between the TL value and the contour value for that band. No credit is given for TL data above the contour.3.Sum the deficiencies and identify the maximum deficiency.4.Increase the trial contour number in 1-dB increments to find the highest-numberedcontour that meets both of the following criteria:a)The sum of the deficiencies is less than or equal to 32 dB, andb)The maximum deficiency in any one band does not exceed 8 dB.5.The resulting value at 500 Hz represents the STC value.R W · · · ISO 717-1 ed. 1 1982 and ISO 717-1 ed. 2 1996ISO European equivalent of the US STC ClassBasically the European approach equals the US approach. The main differences are the fre-quency range. STC covers the range 125 Hz to 4000 Hz, while R W covers 100 Hz to 3150 Hz. So both cover 16 one-third octave bands.STC equals the 500 Hz band of the reference curve with 0 dB, While R W sets the 500 Hz refer-ence value to 52 dB, equaling a standard separation between houses.The calculation methods show that this level doesn't matter. Important is the shape of the reference curve (relative weighing), which are comparable for both standards.ISO 717-1 ed.1 1982 uses the same maximum deficiency 8 dB rule as noted in point 4. bAs such the R W calculation method of this edition equals exactly the principles described in points 1. to 4.ISO 717-1 ed.2 1996 skipped this 8 dB rule, substituting this rule by new single number rat-ings (not covered by this document). The R W however is still used as a standard single num-ber rating, without inclusion this 8 dB rule (meaning old pré-1996 and new RW's calculated on the same TL values can differ from one another).When we should shift this ISO 717-1 R W reference contour one will notice that the contour is exactly similar to the ASTM E413-87 contour with this difference that ISO has an additional value for the 100 Hz band, and one lacking value for the 4000 Hz.Since the lowest frequencies are often the most defining ones in the calculation of a single number rating, the standard European approach is a bit more stringent than the US approach.To calculate the RW,1.Contrary to STC, the TL (in Europe called R) values must be given with an accuracyof 1/10 dB2.Select a trial contour for comparison with the measured TL data.3.Identif y bands with “deficiencies”, where the measured TL data fall below the co n-tour under consideration. The magnitude of the deficiency is the differencebetween the TL value and the contour value for that band. No credit is given for TL data above the contour.4.Sum the deficiencies and identify the maximum deficiency.5.Increase the trial contour number in 1-dB increments to find the highest-numberedcontour that meets both of the following criteria:a)The sum of the deficiencies is less than or equal to 32 dB, andb)The 8 dB rule∙Old ISO 717-1 ed. 1 1982:The maximum deficiency in any one band does not exceed 8 dB.∙New ISO 717-1 ed. 2 1996:The 8 dB rule is skipped all together and substituted by alternative singlenumber correction factors, one for a more accurate calculated dB(A)weighting and a second for traffic/music noise (not covered by this docu-ment).This means one should be careful interpreting existing RW's, since valuesdated before ed. 2 of the standard, cover a bit a different content.6.The resulting value at 500 Hz represents the R W value.MTC: Music/Machinery Transmission ClassClassification for Rating Sound Insulation for music and machineryEven when R W is a bit more stringent than STC, both standards, give a very low relative im-portance in relation to low frequencies, making it a bad approach to rate single number in-sulation values in function of traffic, mechanical noise sources and music.Therefore a new class was designed by H. Stanley Roller, Architectural Construction Man-ager, Acoustics, United States Gypsum Company, in order to compensate for the neglect of the STC Class in view of this low frequent noise.This class however never became an official standard, and most likely never will be.The following is a poetic license by the author as a possible explanation (without knowing the exact history, open for corrective suggestions by readers of this document):Both the European R W and the ASTM STC class are old norms designed in a period, that computers and electronic calculators were still rare objects, hard to get by. That made loga-rithmic calculations, on which most acoustic calculations are based, difficult and time co n-suming, certainly for people the standards where meant for.This resulted in a more graphical approach, only needing arithmetic calculations. In fact from a mathematical/acoustical approach this is a rather inaccurate approach, which is based on empirical statistics. New norms as OITC and lots of others, are much more logical from a mathematical point of view, taking the nowadays calculation means into account. In fact exact logarithmic calculated single number ratings are easier with current calculation means, than the old approach, which is much more complicated to put in simple formulae. The MTC Class is 100% based on an STC calculation, with the same reference spectrum and the same frequency range, but with some added restrictions calculated on the two lowest frequency bands of 125 Hz and 160 Hz.This method was most likely chosen, since it started from a well-known STC calculation pro-cedure, meaning that acceptance of this rather graphical method (complex for easy calcula-tion) could be expected to be good.However the existence now of the later designed OITC class for outdoor noise (with a much more modern acoustic/mathematical approach) will most likely prevent the MTC to become a new official standard. One can expect that new norms will much more resemble the OITC approach.A) To calculate the MTC, one first starts with the standard STC procedure1.Select a trial contour for comparison with the measured TL data.2.Identify bands with “deficiencies”, where the measured TL data fall below the co n-tour under consideration. The magnitude of the deficiency is the difference between the TL value and the contour value for that band. No credit is given for TL data above the contour.3.Sum the deficiencies and identify the maximum deficiency.4.Increase the trial contour number in 1-dB increments to find the highest-numberedcontour that meets both of the following criteria:a)The sum of the deficiencies is less than or equal to 32 dB, andb)The maximum deficiency in any one band does not exceed 8 dB.5.The resulting value at 500 Hz represents the STC value.B) Then one adds the following restrictions/additions to the STC procedure:6.Check if there are deficiencies or surpluses at the 125 Hz and 160 Hz bands (calcu-lated contour versus TL values) after determining the STC contour.a)If you have deficiencies in one or both frequency bands, i.e. the TL is less thanthe contour:i) Lower the contour further until these deficiencies are eliminated.ii)Then read the MTC as the value of the contour at 500 Hz, yielding an MTC that is lower than the STC.b)If after determining the STC, you have surpluses at 125 AND 160 Hz, or sur-pluses at 125 OR 160 Hz while the other value is 0 (meaning contour not low-ered by point 6. a ) the MTC is going to be more than the STC:i) Add the surpluses at 125 and 160 Hz.ii)Divide that sum by 3, and round result to the unit (whole dB's).iii)Add the result to the STC, with this limit that the MTC can never be more than STC plus 4.OITC: Outdoor-Indoor Transmission Class · · ·ASTM E1332-90 (Re-approved 1994) Standard Classification for Determination of Outdoor-Indoor Transmission ClassASTM under the leadership of another USG staff member Keith Walker, did standardize an-other measure:The Outdoor-Indoor Transmission Class (OITC) method assigns a single number rating to measured Sound Transmission Loss (TL) data obtained in accordance with ASTM E-90. The OITC is defined as the A-weighted sound level reduction of a test specimen in the presence of an idealized mixture of transportation noises: aircraft takeoff, freeway, and railroad passby. The rating is computed from measured TL data in one-third octave bands from 80 Hz to 4000 Hz, inclusive.Note from the author: The OITC approach is a modern acoustic/mathematical logic and easy to calculate approach. One can enter the whole standard in one single formula. In fact one can easily substitute the reference spectrum by any custom spectrum covering any frequency range one desires. The level of the spectrum itself doesn't matter since the logarithmic sum factor 100.13 is integrated to correct the formula to a frequency related energetic distribution. In arithmetic terms: the spectrum and its sum is used to recalculate the relative contribution (percentage) of the individual frequency bands, versus a total of 100%.In order to use this approach for other spectra, just enter a dB(A) weighted spectrum, calcu-late the logarithmic sum of this spectrum, and substitute the factor 100.13 by this logarithmic total.To compute the OITC:1. Subtract the measured specimen TL for each one-third octave band from the corre-sponding A-weighted Reference Spectrum for that band. A-weighted Reference Spectrum levels are:1. Perform a logarithmic sum of the one-third octave band results of Step 1,2. The OITC is the difference, rounded to the nearest decibel, between the value 100.13and the logarithmic sum from Step 2. Note this value of 100.13 is the to 2 decimals rounded logarithmic sum of the A-weighted reference spectrumThe entire process can be conveniently expressed in a mathematical equation:where AWRS i is the A-wt. Reference Sound Level and TL i is the Sound Transmission Loss, for each one-third octave band. (The second term of this equation is the logarithmic sum mentioned in Step 2.)Note – Older TL test reports, and TL test reports from some laboratories with smaller reverberation chambers, may not include data for the 80 Hz and 100 Hz bands. Such test reports cannot be used to compute OITC.Note from the Author:While indeed a deviating frequency range does not allow an official OITC value as per the current standard, the method easily allows to adjust the frequency range (explained before in document) resulting in an unofficial single number rating, but which is still much more rep -resentative than the alternative STC rating when low-frequent noise is involved.Remember: One can easily apply the method for specific purposes as e.g. standardized music spectra. ()OITC AWRS i TL i i Hz Hz =-⋅⎧⎨⎩⎫⎬⎭-=∑10013101010804000.log。

python torch小波变换

python torch小波变换Python Torch小波变换小波变换是一种在信号处理和图像处理中广泛应用的数学工具。

它能够将一个信号或图像分解成不同频率的子信号或子图像，并且能够保留原始信号或图像的重要信息。

在本文中，我们将介绍如何使用Python Torch进行小波变换，并且讨论小波变换在图像处理中的应用。

一、小波变换的基本原理小波变换是一种基于函数的变换方法，它通过将函数与一组小波基函数进行卷积运算，将函数在时域和频域中的信息相互转换。

在小波变换中，小波基函数是由一个母小波函数进行平移和伸缩得到的。

小波基函数具有局部性和多分辨率的特点，能够很好地表示信号或图像的局部特征。

二、Python Torch中的小波变换Python Torch是一个基于Python的科学计算包，它提供了丰富的数学函数和工具，方便进行数据处理和模型建立。

在Python Torch 中，我们可以使用torch库中的wavelet函数来进行小波变换。

在使用Python Torch进行小波变换时，我们需要先将信号或图像转换为torch张量。

然后，我们可以使用torch库中的wavelet函数来进行小波变换。

wavelet函数接受两个参数，第一个参数是输入信号或图像的张量，第二个参数是小波基函数的类型。

在torch库中，我们可以选择haar、db、sym、coif、bior、rbio、dmey、gaus、mexh、morl等不同的小波基函数。

三、小波变换在图像处理中的应用小波变换在图像处理中有着广泛的应用。

其中，最常见的应用是图像去噪和图像压缩。

1. 图像去噪小波变换能够将图像分解成不同频率的子图像，其中高频子图像包含了图像中的噪声信息。

通过对高频子图像进行阈值处理，可以将噪声滤除。

然后，再将处理后的子图像进行小波反变换，即可得到去噪后的图像。

2. 图像压缩小波变换能够将图像分解成不同频率的子图像，其中低频子图像包含了图像中的大部分能量信息，而高频子图像包含了图像中的细节信息。

倍频程与十倍频程

将全频域按几何等比级数的间隔划分，使得中心频率fc取做带宽上、下限f1、f2的几何平均值，且带宽h＝f2－f1 总是和中心频率fc保持一常数关系，h＝v×fc。

如果v等于根号二的倒数（0.707)，那么f2＝2f1，则定义这样的频率带宽叫倍频程带宽；如果v等于三倍根号二的倒数（0.236），那么h＝0.236fc，则定义这样的频率带宽为1/3倍频程带宽。

1/3倍频程作用主要是分析噪声能量的频率分布。

另外做分析的时候加了计权网络可起到滤波功能。

每个倍频程或者1/3倍频程的获得是通过带通滤波实现的。

但是作为总的倍频程或者1/3倍频程分析来看，主要是为了研究信号能量在不同频带的分布。

使用1/3倍频程主要是因为人耳对声音的感觉，其频率分辨能力不是单一频率，而是频带，而1/3倍频程曾经被认为是比较符合人耳特性的频带划分方法，不过现在心理声学里提出了Critical Band这么个频带划分方法，听说更符合人耳特性。

先要知道1/3倍频程的划分方法，相关的书和国标都有公式和现成的数据表格，然后，你将时间域的声信号fft变换到频率域，对定义的每个1/3倍频带的声压计算等效连续声压级。

这就是1/3倍频程声压级。

FFT后再进行1/3倍频程分析，在王济和胡晓编“MATLAB在振动信号处理中的应用”（中国水利水电出版社）一书中有一节用介绍1/3倍频程分析，它是在FFT之后用1/3倍频程滤波器对信号进行分析处理，求出1/3倍频程滤波器输出的均方根值，并提供了MATLAB程序。

Spectrum analysis using filters whose bandwidth is a fractional ratio of the center frequency of the filter. For example, a 1/3 octave filter centered at 1000 Hz would have a bandwidth of 260 Hz (26% equals 1/3 octave). Bandwidth (relative to a normalized center frequency of 1) is computed as 2(1/N)-1. The typical bandwidths used (primarily for acoustical and vibration ananlysis) are 1/1 octave, 1/3, 1/12, and 1/24 octave.Octave band filters do not have infinitely steep skirts. Therefore, an isolated tone may produce a reading in adjacent octave bands. Also, a tone at the nominal boundary between two bands produces an equal reading in both. (For example, a 60 dB tone at 707.1 Hz would give readings of 57 dB each in the 500 Hz and 1000 Hz octave bands.) Note also that filters designed according to ANSI S1.11 and IEC 1260 filters have different skirts. Bands adjacent to a strong tone may have different numerical readings with the two types of filters. The actual filter band center frequencies are typically developed as a series of powers of 21/3 times 1000 Hz, and therefore, may not correspond precisely to the nominal band center frequencies.1/N octave filters have a constant relative bandwidth, which means that the Q factor of the filters are the same. In many respects, this is similar to many natural systems, which tend to have a similar behavior and are best viewed on a logarithmic frequency axis. For example, the frequency response of a simple (first order) low-pass filter looks like a straight line when plotted on a logarithmic frequency axis.The following is a table of octave and third-octave filter center frequencies:国外倍频程计算.JPG(44.3 KB, 下载次数: 57)Table below summarized the octave and the 1/3 octave middle frequencies and bands computed from expressions reported in the table above.The figure represents the conversion from narrow frequency bands within the range [200-4000] Hz to one-third octave frequency bands for the sound absorption coefficient of a 30 mm-thick fibrous纤维的material measured at normal incidence法线入射in a standing wave tube. The values of the sound absorption coefficient included in a 1/3 octave band are averaged平均; the obtained mean value of the sound absorption coefficient is then reported at the 1/3 octave middle frequency.频带：把宽广的声音频率变化范围划分为若干较小的段落，叫做频带(频程)。

基于Octave卷积的混合精度神经网络量化方法

2021⁃05⁃10计算机应用,Journal of Computer Applications2021,41(5):1299-1304ISSN 1001⁃9081CODEN JYIIDU http ：//基于Octave 卷积的混合精度神经网络量化方法张文烨1，2*，尚方信2，郭浩2，3（1.中国人民大学信息学院，北京100872；2.山西虚拟现实产业技术研究院有限公司，太原030024；3.太原理工大学信息与计算机学院，太原030024）（∗通信作者电子邮箱slicent@ ）摘要：浮点数位宽的深度神经网络需要大量的运算资源，这导致大型深度神经网络难以在低算力场景（如边缘计算）上部署。

为解决这一问题，提出一种即插即用的神经网络量化方法，以压缩大型神经网络的运算成本，并保持模型性能指标不显著下降。

首先，基于Octave 卷积将输入特征图的高频和低频成分进行分离；其次，分别对高低频分量应用不同位宽的卷积核进行卷积运算；第三，使用不同位宽的激活函数将高低频卷积结果量化至相应位宽；最后，混合不同精度的特征图来获得该层卷积结果。

实验结果证实了所提方法压缩模型的有效性，在CIFAR -10/100数据集上，将模型压缩至1+8位宽时，该方法可保持准确率指标的下降小于3个百分点；在ImageNet 数据集上，使用该方法将ResNet50模型压缩至1+4位宽时，其正确率指标仍高于70%。

关键词：深度神经网络；模型量化；模型压缩；卷积神经网络；深度学习中图分类号：TP391.41文献标志码：AMixed precision neural network quantization method based on Octave convolutionZHANG Wenye 1，2*，SHANG Fangxin 2，GUO Hao 2，3（1.School of Information ，Renmin University of China ，Beijing 100872，China ；2.Shanxi Extended Reality Industrial Technology Research Institute Company Limited ，Taiyuan Shanxi 030024，China ；3.College of Information and Computer ，Taiyuan University of Technology ，Taiyuan Shanxi 030024，China ）Abstract:Deep neural networks with 32-bit weights require a lot of computing resources ，making it difficult for large -scale deep neural networks to be deployed in limited computing power scenarios （such as edge computing ）.In order to solve this problem ，a plug -and -play neural network quantification method was proposed to reduce the computational cost of large -scale neural networks and keep the model performance away from significant reduction.Firstly ，the high -frequency and low -frequency components of the input feature map were separated based on Octave convolution.Secondly ，the convolution kernels with different bits were respectively applied to the high -and low -frequency components for convolution operation.Thirdly ，the high -and low -frequency convolution results were quantized to the corresponding bits by using different activation functions.Finally ，the feature maps with different precisions were mixed to obtain the output of the layer.Experimental results verify the effectiveness of the proposed method on model compression.When the model was compressed to 1+8bit （s ），the proposed method had the accuracy dropped less than 3percentage points on CIFAR -10/100dataset ；moreover ，the proposed method made the ResNet50structure based model compressed to 1+4bit （s ）with the accuracy higherthan 70%on ImageNet dataset.Key words:deep neural network;model quantification;model compression;convolutional neural network;deeplearning引言近年来，卷积神经网络（Convolutional Neural Network ，CNN ）被成功应用于许多计算机视觉问题的解决上，例如图像分类［1-2］、目标检测［3］和实例分割［4］等。

数据通信原理实验指导书

实验一编码与译码一、实验学时：2学时二、实验类型：验证型三、实验仪器：安装Matlab软件的PC机一台四、实验目的：用MATLAB仿真技术实现信源编译码、过失操纵编译码,并计算误码率。

在那个实验中咱们将观看到二进制信息是如何进行编码的。

咱们将要紧了解：1．目前用于数字通信的基带码型2．过失操纵编译码五、实验内容：1．经常使用基带码型（1）利用MATLAB 函数wave_gen 来产生代表二进制序列的波形,函数wave_gen 的格式是：wave_gen(二进制码元,‘码型’,Rb)此处Rb 是二进制码元速度,单位为比特/秒（bps）。

产生如下的二进制序列：>> b = [1 0 1 0 1 1];利用Rb=1000bps 的单极性不归零码产生代表b的波形且显示波形x，填写图1-1：>> x = wave_gen(b,‘unipolar_nrz’,1000);>> waveplot(x)（2）用如下码型重复步骤（1）（提示：能够键入“help wave_gen”来获取帮忙），并做出相应的记录：a 双极性不归零码b 单极性归零码c 双极性归零码d 曼彻斯特码(manchester)x 10-3x 10-3图1-1 单极性不归零码图1-2双极性不归零码x 10-3x 10-32．过失操纵编译码（1）利用MATLAB 函数encode 来对二进制序列进行过失操纵编码, 函数encode 的格式是：A ．code = encode(msg,n,k,'linear/fmt',genmat)B ．code = encode(msg,n,k,'cyclic/fmt',genpoly)C ．code = encode(msg,n,k,'hamming/fmt',prim_poly)其中A ．用于产生线性分组码，B ．用于产生循环码，C ．用于产生hamming 码，msg 为待编码二进制序列，n 为码字长度，k 为分组msg 长度，genmat 为生成矩阵，维数为k*n ，genpoly 为生成多项式,缺省情形下为cyclpoly(n,k)。

倍频程与十倍频程

1/3倍频程作用主要是分析噪声能量的频率分布。

另外做分析的时候加了计权网络可起到滤波功能。

每个倍频程或者1/3倍频程的获得是通过带通滤波实现的。

但是作为总的倍频程或者1/3倍频程分析来看，主要是为了研究信号能量在不同频带的分布。

这就是1/3倍频程声压级。

python实现基于短时傅里叶变换的谱峭度算法

在介绍python实现基于短时傅里叶变换的谱峭度算法之前，我们需要先了解一些基本概念。

短时傅里叶变换（STFT）是一种在信号处理和分析中常用的方法，用于将一个信号分解成时间和频率的分量。

谱峭度算法是一种用于描述信号局部特性的算法，它可以帮助我们更好地理解信号的频谱特性。

1. 了解短时傅里叶变换（STFT）短时傅里叶变换是离散傅里叶变换（DFT）的一种变种，它将信号分成多个时间窗口，在每个窗口上进行傅里叶变换，以获得时间和频率上的信号特性。

STFT常用于分析非稳态信号，可以帮助我们理解信号在不同时间段内的频率谱特性。

2. 理解谱峭度算法谱峭度算法是一种用于描述信号频谱局部特性的指标，它可以帮助我们发现信号中的尖锐或奇怪特性。

谱峭度算法通常通过计算信号频谱的尖峰度来描述信号的局部特性，尖峰度越高，表示信号在该频率处的局部特性越强烈。

3. Python实现基于短时傅里叶变换的谱峭度算法在Python中，我们可以利用第三方库scipy来实现基于短时傅里叶变换的谱峭度算法。

我们需要使用scipy库中的signal模块来进行短时傅里叶变换，然后利用scipy库中的spectral模块来计算信号的谱峭度。

通过这些功能，我们可以很容易地对信号进行频谱分析，并计算出其局部特性。

4. 个人观点和理解对于短时傅里叶变换和谱峭度算法，我个人认为它们在信号处理和分析中有着非常重要的应用价值。

使用这些算法，我们可以更深入地理解信号的频谱特性，发现其中的局部特性，并为后续的信号处理和分析工作提供重要参考。

总结和回顾通过本文的介绍，我们了解了短时傅里叶变换和谱峭度算法的基本概念，以及在Python中如何实现基于短时傅里叶变换的谱峭度算法。

这些内容对于我们进一步深入学习信号处理和频谱分析都具有重要意义。

我在文章中多次提及了python实现基于短时傅里叶变换的谱峭度算法，并按照指定的要求进行了深度和广度兼具的探讨。

希望这篇文章能够帮助你更好地理解这一主题！短时傅里叶变换（STFT）在信号处理和分析中有着广泛的应用，特别是当信号是非稳态的时候。

频程谱总级值

频程谱总级值
频程谱总级值（Octave Band Level）是一个用于描述声音或振动在不同频率范围内能量分布的指标。

它通常用于环境噪声测量、振动分析、声学设计等领域。

频程谱总级值是将整个频率范围划分为若干个频程（Octave Bands），然后计算每个频程内的声压级或振动级，最后将所有频程的声压级或振动级求和得到的一个总级值。

由于人耳对不同频率的声音敏感度不同，因此频程谱总级值能够更好地反映人对声音的主观感受。

计算频程谱总级值的步骤如下：
１．确定频率范围，并将其划分为若干个频程。

通常，每个频程的中心频率与上下限频率的比值为2:1，因此称为“频程”。

２．在每个频程内测量声压级或振动级。

声压级或振动级通常使用分贝（dB）作为单位。

３．将所有频程的声压级或振动级求和，得到频程谱总级值。

需要注意的是，频程谱总级值受到测量环境、测量仪器、测量方法等多种因素的影响，因此在实际应用中需要进行合理的测量和数据处理。

同时，不同国家和地区也可能采用不同的频程划分方法和计算标准，因此在比较不同数据时需要注意统一标准。

Python与Octave的交互

Python与Octave的交互Python与Octave的交互随着计算机技术的不断发展，人们的生活和工作方式也在不断发生变化。

作为一种非常流行的计算机编程语言，Python已经被广泛应用于数据分析、机器学习、人工智能等领域。

作为一种数值计算软件，Octave也被广泛应用于科学计算、数据分析等领域。

本文将介绍Python与Octave的交互方式，并探讨如何使用这种交互方式来提高工作效率。

Python与Octave的交互方式Python与Octave之间的交互有多种方式，这里主要介绍以下几种常用的方式。

1.通过命令行交互这种方式比较简单，只需要在Python命令行或者Octave命令行中输入对应的命令即可实现交互。

例如，我们可以在Python命令行中输入以下命令：import osos.system('octave --eval "x=[1 2 3];disp(x);"')这个命令会启动Octave并执行一个矩阵赋值的操作，然后输出矩阵x的值。

通过这种方式，Python和Octave之间可以非常灵活地交互，可以实现各种复杂的计算操作。

但是，通过命令行交互的方式存在一些不方便之处，例如输入的命令比较长，不易读取和编辑；命令的执行结果也不太方便保存和处理等。

因此，更常用的交互方式是通过Python或Octave中的扩展模块实现。

2.通过Python的oct2py模块oct2py是一个Python的扩展模块，可以实现Python与Octave之间的交互。

使用oct2py模块可以让Python和Octave之间实现更加方便、直观的交互操作。

首先需要安装oct2py模块，在Python命令行中输入以下命令：pip install oct2py安装完成后，可以在Python代码中使用以下语句来启动Octave 并进行计算：from oct2py import octavex = octave.eval('sin(2*pi*0.05*(1:100));')print(x)这个例子中，我们使用octave.eval()函数来执行一段Octave代码，这段代码的作用是生成一个包含100个正弦波的向量。

octave的基本操作

octave的基本操作Octave是一种开源的数值计算软件，它提供了一种灵活高效的方式来进行数值计算和数据分析。

本文将介绍Octave的基本操作，包括变量的定义、矩阵运算、函数的使用以及图形绘制等。

1. 变量的定义在Octave中，可以使用等号来定义变量。

例如，可以使用以下语句将一个常数赋值给变量x：```octavex = 10;```变量的命名规则与其他编程语言类似，可以使用字母、数字和下划线，但不能以数字开头。

2. 矩阵运算Octave中的矩阵运算非常方便。

可以使用方括号来定义矩阵，使用乘法运算符来进行矩阵乘法。

例如，可以使用以下语句定义一个2行3列的矩阵A，并计算矩阵A与向量x的乘积：```octaveA = [1, 2, 3; 4, 5, 6];y = A * x;```除了乘法运算，Octave还支持矩阵的转置、逆矩阵和特征值等运算。

3. 函数的使用Octave提供了许多数学函数和统计函数，可以方便地进行数值计算和数据分析。

例如，可以使用以下语句计算一个向量的平均值和标准差：```octavev = [1, 2, 3, 4, 5];mean_value = mean(v);std_value = std(v);```除了内置函数，Octave还支持用户自定义函数的编写和调用。

4. 图形绘制Octave提供了丰富的绘图函数，可以用于绘制曲线、散点图、柱状图等。

例如，可以使用以下语句绘制一个正弦曲线：```octavex = linspace(0, 2*pi, 100);y = sin(x);plot(x, y);```除了基本的绘图函数，Octave还支持图形的自定义设置，如添加标题、轴标签、图例等。

5. 文件的读写Octave可以读写各种常见的文件格式，如文本文件、CSV文件和MAT文件等。

例如，可以使用以下语句将一个矩阵写入到CSV文件中：```octaveA = [1, 2, 3; 4, 5, 6];csvwrite("data.csv", A);```可以使用以下语句从CSV文件中读取数据并存入一个矩阵：```octaveB = csvread("data.csv");```除了CSV文件，Octave还支持其他常见的文件格式，如Excel文件和图像文件等。

Waves GEQ用户指南说明书

WAVESGEQ GRAPHIC EQUALIZERUSER GUIDETABLE OF CONTENTSCHAPTER 1 – INTRODUCTION (3)1.1W ELCOME (3)1.2P RODUCT O VERVIEW (3)1.3C ONCEPTS AND T ERMINOLOGY (4)1.4C OMPONENTS (6)CHAPTER 2 – QUICK START GUIDE (7)CHAPTER 3 – INTERFACE AND CONTROLS (9)3.1I NTERFACE (9)3.2C ONTROLS (10)CHAPTER 4 – THE WAVESYSTEM (17)4.1T HE W AVE S YSTEM T OOLBAR (17)4.2P RESET H ANDLING (17)4.3I NTERFACE C ONTROLS (20)4.4W AVES P REFERENCES (P RO T OOLS ONLY) (22)Chapter 1 – Introduction1.1 WelcomeThank you for choosing Waves! In order to get the most out of your Waves plugins, please take the time to read through this manual.In conjunction, we also suggest that you become familiar with . There you will find an extensive Answer Base, the latest Tech Specs, detailed Installation guides, new Software Updates, and current information on Authorization and Registration.By signing up at , you will receive personalized information on your registered products, reminders when updates are available, and information on your authorization status.1.2 Product OverviewWaves GEQ is a full-featured graphic equalizer with both Classic and Modern mono and stereo components, featuring 30 ISO bands plus high and low pass filters, a high precision floating parametric EQ bell filter, and a real-time analyzer.GEQ Classic components use traditional proportional Q filters inspired by the renowned DN series 1/3 octave equalizers, which narrow the filter width as you increase a band’s gain; GEQ Modern components utilize special Flat-Top filters which eliminate the artifacts associated with band interaction, and provide perfect plateau and stair-stepped responses. Set band gains one-by-one, or draw your curve via touch-screen or mouse. Finally, GEQ’s integrated real-time analyzer lets you compare the difference between two sources. Created for live sound but equally useful in the studio as well, GEQ is powerful proof that all equalizers are not created equal.Waves GEQWaves GEQ1.3 Concepts and TerminologyGraphic EQMost graphic equalizers consist of a bank of filters with pre-determined (fixed) cutoff frequencies, typically divided into bands of 1/3 or one octave each, with frequency cutoffs defined by ISO standards (International Organization for Standardization). It’s common to see the gain controls set as a horizontal array of faders with the lowestfrequency on the left and the highest on the right. So if one were to draw a line that plots the gain fader positions at any given time, the resultant line would be a graphicalrepresentation of the equalizer’s current frequency response, hence the name “graphic equalizer.”The GEQ offers three bands per octave for a total of 30 bands, similar to the hardware devices commonly used in live sound reinforcement systems. GEQ includes a faders array as well as parametric graph, plus a real-time analyzer with flexible routing.Proportional QFor its 30 main bands, the GEQ Classic component uses “Proportional Q” filters. The Q value is proportional to the gain adjustment; increasing a band’s gain narrows the filter width.Waves GEQFlat-Top FiltersThe GEQ Modern component utilizes special Flat-Top filters which eliminate the artifacts associated with band interaction, and provide perfect plateau and stair-steppedresponses. Thus, boosting a set of consecutive bands by 6 dB will result in a 6 dB boost plane, without additional boost due to band interaction.Band InteractionClose to 0 dB, Proportional Q bells have a fairly wide frequency range. This is not an issue when boosting or cutting frequencies in different ranges; however, when boosting adjacent bands, the two filters will overlap and interact, resulting in unintended gain levels.Classic Proportional Q Filters Modern Flat-Top Filters1.4 ComponentsWaveShell technology enables us to split Waves processors into smaller plugins, which we call components. Having a choice of components for a particular processor gives you the flexibility to choose the configuration best suited to your material.GEQ has a total of four components:•GEQ Classic Mono•GEQ Classic Stereo•GEQ Modern Mono•GEQ Modern StereoWaves GEQChapter 2 – Quick Start GuideUsing the EQ1. Open a mono or stereo GEQ component on a track.2. Select Drag or Draw mode.3. Drag mode: Select individual band faders, move each up or down to set itsboost/cut.Draw mode: Select a band fader, move up or down and continuously left or right to draw the EQ curve.4. For further adjustments, use the floating Parametric EQ section to set non-ISOfrequency cutoffs.Setting and Calibrating the Analyzer1. Open a mono or stereo GEQ component on an output track.2. On the WaveSystem toolbar, go to RTA1 and select a source. (See Section3.2.)3. On the WaveSystem toolbar, go to RTA2 and select a sidechain input. Forexample, the sidechain input can receive its signal from a microphone whichmeasures the RTA1 source after passing through the PA system:4. On the WaveSystem toolbar, activate Calibration Mode by clicking Calibration.5. Select the RTA Mode: Fast or Slow.6. If required, calculate the delay between the two RTA input sources, and enter thevalue in milliseconds into the RTA1 Delay field.Waves GEQ7. Adjust the RTA1 and RTA2 displayed curve levels using the faders next to theinput and output meters. Please note: Adjusting these faders will affect only the analyzer; it will not affect your output.8. Press Diff to view the difference in frequency response between RTA1 andRTA2.9. Match the two signals manually using the EQ section of the plugin.Waves GEQChapter 3 – Interface and Controls3.1 InterfaceGEQ Classic StereoGEQ Modern StereoWaves GEQWaves GEQ3.2 ControlsAnalyzer SectionGRAPHIC DISPLAY / ANALYZER shows the EQ curve, RTA1 and RTA2 input RMS frequency responses and the Difference between them.Brown EQ curve on mono components; Left channel EQ curve on stereo components. Blue Right channel EQ curveYellow RTA1 input frequency responseCyan RTA2 input frequency responseRed Difference between RTA1 and RTA2Frequency Range: 20 – 20,000 HzLevel Range: -80 – 0 dBFSINPUT METER displays input level (peak) in dBFS.Range: -80 – 0 dBFSOUTPUT METER displays output level (peak) in dBFS.Range: -80 – 0 dBFSRTA1 assigns the first input to the real-time analyzer.RTA2 assigns the second input to the real-time analyzer.Mono Component Range: Off, In, SC-In, OutStereo Component Range: Off, L-In, R-In, LSC-In, RSC-In, L-Out, R-Out, L+RDIFFERENCE mode turns off the real-time analyzer and displays the difference in frequency response between RTA1 and RTA2. This is helpful when you need to:▪Adjust the output signal in relation to the measured post-system response.▪Match the left and right frequency responses.Range: On, OffWaves GEQCalibration ModeCALIBRATION activates the Calibration mode which calibrates the analyzer.RTA1 & RTA2 FADERS are used to adjust and match the analyzer input levels.RTA CALIBRATION set the response time of the analyzer.Please note: When Difference is on, the response is Slow, regardless of the RTA Calibration setting.Range: Fast, SlowRTA1 DELAY sets the alignment of RTA1 to RTA2.Range: 0 – 341 millisecondsWaves GEQGraphic EQ SectionSCALE controls the maximum range of GEQ faders. The scale indicators next to the band faders update according to the selected scale. For example, if the 6 dB scale is selected and 25 Hz is set to +5 dB, changing the scale to 12 dB increases the boost at 25 Hz to +10 dB.Range: 6, 12, 18 dBQ functions as a multiplier for the proportional Q algorithm. (Classic components only) Range 0.250 – 4.000Waves GEQISO FADERS control the gain of selected frequency centers.Gain Range: -18 to +18 (dependent on Scale settings)Frequency Cutoffs: 25 Hz, 31 Hz, 40 Hz, 50 Hz, 63 Hz, 80 Hz, 100 Hz, 125 Hz, 160 Hz, 200 Hz, 250 Hz, 315 Hz, 400 Hz, 500 Hz, 630 Hz, 800 Hz, 1 kHz, 1.25 kHz, 1.6 kHz, 2 kHz, 2.5 kHz, 3.15 kHz, 4 kHz, 5 kHz, 6.3 kHz, 8 kHz, 10 kHz, 12.5 kHz 16 kHz, 20 kHzDRAG selects the method of setting the band faders.▪Drag mode: Select individual band faders; move each up or down to set its boost/cut.▪Draw mode: Select a band fader; move up or down, left or right, continuously, to draw the EQ curve.Range: Drag, DrawLINK relatively links left and right channels, including all bands, high and low pass filters, floating Parametric Bell EQ and Master Gain settings. (Stereo components only) Range: On, OffFLAT snaps all bands to 0 dB.Please note: This does not affect the floating Parametric EQ section.Waves GEQFloating Parametric EQ SectionHP activates the high pass filter.Range: On, OffHP FREQUENCY sets the cutoff frequency of the high pass filter.Range: 20 – 990 HzLP activates the low pass filter.Range: On, OffLP FREQUENCY sets the cutoff frequency of the low pass filter.Range: 1000 – 20,000 HzPARAMETRIC BELL activates the floating parametric bell filter.Range: On, OffPARAMETRIC BELL FREQUENCY sets the cutoff frequency of the floating parametric bell filter.Range 20 – 20,000 HzPARAMETRIC BELL GAIN controls the gain of the floating parametric bell filter. Range: +/- 18 dBWaves GEQPARAMETRIC BELL Q determines the width of the floating parametric bell filter. Range: 0.250-50MASTER GAIN controls the output level of the entire plugin.Range: +/- 18 dBWaves GEQChapter 4 – The WaveSystem4.1 The WaveSystem ToolbarAll Waves plugins feature the WaveSystem toolbar which takes care of most administrative functions you will encounter while working with your Waves software. The features of the WaveSystem toolbar are the same on practically all Waves plugins, so familiarity with its features will be helpful whichever plugin you are using.Toolbar FunctionsOpens the plugin About boxUndo Undoes the last 32 actionsRedo Redoes the last 32 undone actionsSetup A/B Toggles between two presets, useful for comparison of parametersettingsL/R Arrows Move to the previous or next presetCopy A→B Copies the current settings to the second preset registerLoad Recalls presets from fileSave Saves presets in the Waves file formatsOpens the PDF manual for the plugin you are using4.2 Preset HandlingPreset TypesFactory Presets are permanent presets in the Load menu. Factory presets cannot be overwritten or deleted. When applicable, different component plugins may have different factory presets.User Presets are your favorite settings of the plugin saved as a preset in the Load menu, under ‘User Presets’. User Presets can be overwritten and deleted.Waves GEQSetup Files may contain more than one preset. For example, a single file can contain all the presets for a session. When you open a Setup File, all its setups become part of your Load pop-up menu for fast access. This can be particularly useful with multiple instances of a plugin in a single session. By saving all the settings you create into a single Setup File, they can all be quickly available for every instance of that plugin.Loading Presets and SetupsClick on the Load button to see the Load pop-up menu. The menu is divided into four sections. If a section is not currently available it will not appear in the Load pop-up menu.Open Preset File…Select to open any setup or preset file, whether from the Library oryour own creations.‘Filename.xps’: Displays any currently loaded Setup File and its presets.Factory Presets:Displays the default Factory Presets.User Presets:Displays any loaded User Presets.Saving Presets and SetupsClick on the Save button to see the Save pop-up menu. Four options are available. If an option is not currently available it will be grayed out and inaccessible.Save to New File…Select this to start a new Setup file. There are twoprompts - first for the setup filename, then for thepreset name. You must provide a name for both thesetup file and the preset. Click OK (ENTER) tocomplete the save. It is a good idea to create afolder in which to save several setup files for aproject.Save ‘File Name’ – “Preset Name” Overwrites the settings of the loaded preset(whether a User Preset or a preset from a SetupFile) with the current settings. If a Setup File isWaves GEQcurrently loaded, the name of the Setup File isdisplayed followed by the name of the preset itself.If a User Preset is loaded, its name is displayed. Save to ‘File Name’ As…Saves the current settings as a new preset into theSetup file that is open (if one is not open, the optionis grayed out). You will be prompted to give thepreset a name.Put into Preset Menu As…Save the current settings into a User Preset thatwill always be in your Load menu (until deleted).You will be prompted to give this preset a name.User Presets are stored in the plugin’s preferencefile.Deleting PresetsYou may delete User Presets and presets within a Setup File. Factory Presets and Setup Library files cannot be deleted or overwritten.1. Hold the Command (Mac)/Control (PC) key down.2. Click-and-hold the Load button to see the pop-up menu.3. While still holding the Command/Control key, select the preset or setup to delete.4. A confirmation box will appear, allowing you to cancel or ‘OK’ the deletion.A/B Comparison and CopyingThe Setup A/Setup B button may be clicked to compare two settings. If you load a preset in the Setup B position, this will not affect the preset loaded into the Setup A position, and vice-versa.If you want to slightly modify the settings in Setup A, you can copy them to Setup B by clicking on the Copy to B button, then alter Setup A and compare with the original Setup B.Waves GEQThe name of the current setup will be shown in the title bar (on platforms which support it), and will switch as you change from Setup A to Setup B.Note: an asterisk will be added to the preset name when a change is made to the preset.4.3 Interface ControlsControls can be in one of three states:•Not Selected where the control is not the target of any user entry•Selected where the control is the target of mouse control entry only•Selected and Active where the control is the target for both mouse and keyboard entryToggle ButtonsToggle buttons display the state of a control, and allow switching between two or more states. Single-click to change the control’s state. Some toggle buttons have a text display which updates with the current setting, and others (bypass, solo, or monitoring toggles) illuminate when the control is active.Some plugins have link buttons between a pair of toggle buttons, allowing click-and-drag adjustment while retaining the offset between the controls.Value Window ButtonsValue windows display the value of a control and allow click-and-drag adjustment, or direct control via the keyboard.1. Using the mouse, click-and-drag on the value window to adjust. Some valuewindows support left/right, some up/down (as you hover over a button, arrowswill appear to let you know which direction of movement that button supports).You may also use your mouse-wheel to adjust parameter values.2. Using the arrow keys, click once with mouse to select the button, and then useup/down – left/right (depending on the direction supported by that button) toWaves GEQmove in the smallest incremental steps across the button’s range (holding down the arrow keys will move faster through the range).3. Using key entry, double click on the button to open the value window, anddirectly enter the value from your keyboard. If you enter an out of range number, the button stays selected but remains at the current setting. (System beeps ifsystem sounds are on.)Some plugins have link buttons between a pair of value windows, allowing click-and-drag adjustment while retaining the offset between the controls.SlidersClick or scroll the mouse-wheel on the slider itself or anywhere within the sliders track. The numerical value of the slider settings is displayed in a hover window above the slider path.Hover BoxHovering boxes will appear and display the control value when hovering with the mouse over the control.Multiple Control SelectionOne of the most powerful features of the WaveSystem is the ability to select and adjust multiple controls simultaneously. Using the mouse, drag-select the desired group of buttons or graphic controls by clicking and holding at a point outside the controls, and forming a rectangle that includes the controls you wish to adjust. Alternatively, press and hold Shift while clicking the mouse on any control you wish to link. This method is useful when you want to select two or more controls that are not adjacent to one another.Waves GEQTAB FunctionsTAB moves the ‘selected’ status to the next control, with shift-TAB moving in the reverse direction.Additionally, the Mac has an option-TAB function for ‘down’ movement and shift-option-TAB for ‘up’ movement where applicable.If you have several Value Window Buttons selected, TAB functions will take you through the selected controls only.Hitting Esc or Return will return the 'focus' to the DAW application.4.4 Waves Preferences (Pro Tools only)When launching Pro Tools, hold Shift to view the Waves plugin Preferences window. The following options are available:•Don't use AudioSuite plugins•Don’t use RTAS plugins•Rescan all plugins•HUI control surface support (low resolution)•Enable single-click text entryWaves GEQ。

A-weighting

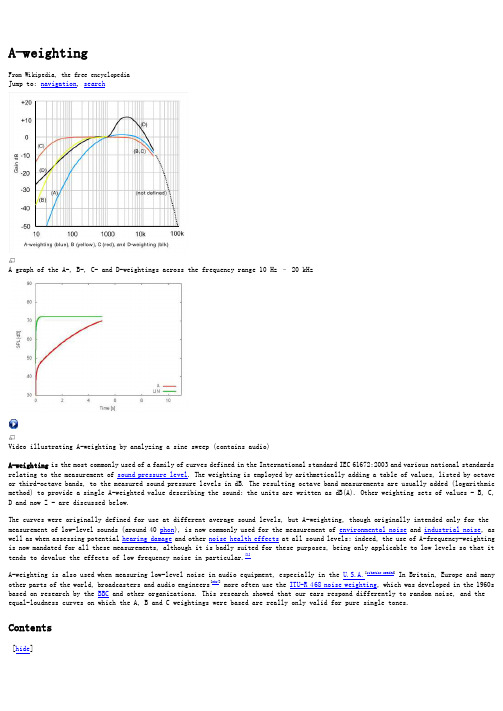
A-weightingFrom Wikipedia, the free encyclopediaJump to: navigation, searchA graph of the A-, B-, C- and D-weightings across the frequency range 10 Hz – 20 kHzVideo illustrating A-weighting by analyzing a sine sweep (contains audio)A-weighting is the most commonly used of a family of curves defined in the International standard IEC 61672:2003 and various national standards relating to the measurement of sound pressure level. The weighting is employed by arithmetically adding a table of values, listed by octave or third-octave bands, to the measured sound pressure levels in dB. The resulting octave band measurements are usually added (logarithmic method) to provide a single A-weighted value describing the sound; the units are written as dB(A). Other weighting sets of values - B, C, D and now Z - are discussed below.The curves were originally defined for use at different average sound levels, but A-weighting, though originally intended only for the measurement of low-level sounds (around 40 phon), is now commonly used for the measurement of environmental noise and industrial noise, as well as when assessing potential hearing damage and other noise health effects at all sound levels; indeed, the use of A-frequency-weighting is now mandated for all these measurements, although it is badly suited for these purposes, being only applicable to low levels so that it tends to devalue the effects of low frequency noise in particular.[1]A-weighting is also used when measuring low-level noise in audio equipment, especially in the U.S.A.[citation needed] In Britain, Europe and many other parts of the world, broadcasters and audio engineers[who?]more often use the ITU-R 468 noise weighting, which was developed in the 1960s based on research by the BBC and other organizations. This research showed that our ears respond differently to random noise, and the equal-loudness curves on which the A, B and C weightings were based are really only valid for pure single tones.Contents[hide]∙ 1 History of A-weighting∙ 2 Deficiencies of A-weighting∙ 3 B-, C-, D- and Z-weightings∙ 4 Environmental noise measurement∙ 5 Audio reproduction and broadcasting equipment∙ 6 Function realisation of some common weightingso 6.1 Ao 6.2 Bo 6.3 Co 6.4 D∙7 Transfer function equivalento7.1 Ao7.2 Bo7.3 Co7.4 D∙8 See also∙9 References∙10 External links[edit] History of A-weightingA-weighting began with work by Fletcher and Munson which resulted in their publication, in 1933, of a set of equal-loudness contours. Three years later these curves were used in the first American standard for sound level meters.[1]This ANSI standard, later revised as ANSI S1.4-1981, incorporated B-weighting as well as the A-weighting curve, recognising the unsuitability of the latter for anything other than low-level measurements. But B-weighting has since fallen into disuse. Later work, first by Zwicker and then by Schomer, attempted to overcome the difficulty posed by different levels, and work by the BBC resulted in the CCIR-468 weighting, currently maintained as ITU-R 468 noise weighting, which gives more representative readings on noise as opposed to pure tones.[citation needed][edit] Deficiencies of A-weightingA-weighting is only really valid for relatively quiet sounds and for pure tones as it is based on the 40-phon Fletcher-Munson curves which represented an early determination of the equal-loudness contour for human hearing.Because of perceived discrepancies between early and more recent determinations, the International Organization for Standardization (ISO) recently revised its standard curves as defined in ISO 226, in response to the recommendations of a study coordinated by the Research Institute of Electrical Communication, Tohoku University, Japan. The study produced new curves by combining the results of several studies, by researchers in Japan, Germany, Denmark, UK, and USA. (Japan was the greatest contributor with about 40% of the data.) This has resulted in the recent acceptance of a new set of curves standardized as ISO 226:2003. The report comments on the surprisingly large differences, and the fact that the original Fletcher-Munson contours are in better agreement with recent results than the Robinson-Dadson, which appear to differ by as much as 10–15 dB especially in the low-frequency region, for reasons that are not explained. Fortuitously, the 40-Phon Fletcher-Munson curve is particularly close to the modern ISO 226:2003 standard.[2]Nevertheless, it will be noted that A-weighting would be a better match to the loudness curve if it fell much more steeply above 10 kHz, and it is likely that this compromise came about because steep filters were difficult to construct in the early days of electronics.[citation needed] Nowadays, no such limitation need exist, as demonstrated by the ITU-R 468 curve. If A-weighting is used without further band-limiting it is possible to obtain different readings on different instruments when ultrasonic, or near ultrasonic noise is present. Accurate measurements therefore require a 20 kHz low-pass filter to be combined with the A-weighting curve in modern instruments. This is defined in IEC 61012 as AU weighting and while very desirable, is rarely fitted to commercial sound level meters.A new technology sound level meter complying with IEC 61672 : 2003[edit] B-, C-, D- and Z-weightingsA-frequency-weighting is mandated by the international standard IEC 61672 to be fitted to all sound level meters. The old B- andD-frequency-weightings have fallen into disuse, but many sound level meters provide for C frequency-weighting and its fitting is mandated — at least for testing purposes — to precision (Class one) sound level meters. D-frequency-weighting was specifically designed for use when measuring high level aircraft noise in accordance with the IEC 537 measurement standard. The large peak in the D-weighting curve is not a feature of the equal-loudness contours, but reflects the fact that humans hear random noise differently from pure tones, an effect that is particularly pronounced around 6 kHz. This is because individual neurons from different regions of the cochlea in the inner ear respond to narrow bands of frequencies, but the higher frequency neurons integrate a wider band and hence signal a louder sound when presented with noise containing many frequencies than for a single pure tone of the same pressure level.[citation needed] Following changes to the ISO standard, D-frequency-weighting should now only be used for non-bypass engines and as these are not fitted to commercial aircraft —but only to military ones — A-frequency-weighting is now mandated for all civilian aircraft measurements.Z- or ZERO frequency-weighting was introduced in the International Standard IEC 61672 in 2003 and was intended to replace the "Flat" or "Linear" frequency weighting often fitted by manufacturers. This change was needed as each sound level meter manufacturer could choose their own low and high frequency cut-offs (–3dB) points, resulting in different readings, especially when peak sound level was being measured. As well, the C-frequency-weighting, with –3dB points at 31.5Hz and 8 kHz did not have a sufficient bandpass to allow the sensibly correct measurement of true peak noise (Lpk)B- and D-frequency-weightings are no longer described in the body of the standard IEC 61672 : 2003, but their frequency responses can be found in the older IEC 60651, although that has been formally withdrawn by the International Electro-technical Commission in favour of IEC 61672 : 2003. The frequency weighting tolerances in IEC 61672 have been tightened over those in the earlier standards IEC 179 and IEC 60651 and thus instruments complying with the earlier specifications should no longer be used for legally required measurements.[edit] Environmental noise measurementA-weighted decibels are abbreviated dB(A) or dBA. When acoustic (calibrated microphone) measurements are being referred to, then the units used will be dB SPL referenced to 20 micropascals = 0 dB SPL. dBrn adjusted is a synonym for dBA.The A-weighting curve has been widely adopted for environmental noise measurement, and is standard in many sound level meters. The A-weighting system is used in any measurement of environmental noise (examples of which include roadway noise, rail noise, aircraft noise). A-weighting is also in common use for assessing potential hearing damage caused by loud noise.A-weighted SPL measurements of noise level are increasingly found on sales literature for domestic appliances such as refrigerators, freezers and computer fans. Although the threshold of hearing is theoretically around 0 dB SPL, sound at this level is practically inaudible and appliances are more likely to have noise levels of 30 to 40 dB SPL.[edit] Audio reproduction and broadcasting equipmentAlthough the A-weighting curve, in widespread use for noise measurement, is said to have been based on the 40-phon Fletcher-Munson curve, research in the 1960s demonstrated that determinations of equal-loudness made using pure tones are not directly relevant to our perception of noise.[3]This is because the cochlea in our inner ear analyses sounds in terms of spectral content, each 'hair-cell' responding to a narrow band of frequencies known as a critical band. The high-frequency bands are wider in absolute terms than the low frequency bands, and therefore 'collect' proportionately more power from a noise source. However, when more than one critical band is stimulated, the outputs of the various bands are summed by the brain to produce an impression of loudness. For these reasons equal-loudness curves derived using noise bands show an upwards tilt above 1 kHz and a downward tilt below 1 kHz when compared to the curves derived using pure tones.This enhanced sensitivity to noise in the region of 6 kHz became particularly apparent in the late 1960s with the introduction of compact cassette recorders and Dolby-B noise reduction. A-weighted noise measurements were found to give misleading results because they did not give sufficient prominence to the 6 kHz region where the noise reduction was having greatest effect, and did not sufficiently attenuate noise around 10 kHz and above (a particular example is with the 19 kHz pilot tone on FM radio systems which, though usually inaudible is not sufficiently attenuated by A-weighting, so that sometimes one piece of equipment would even measure worse than another and yet sound better, because of differing spectral content.ITU-R 468 noise weighting was therefore developed to more accurately reflect the subjective loudness of all types of noise, as opposed to tones.[citation needed] This curve, which came out of work done by the BBC Research Department, and was standardised by the CCIR and later adopted by many other standards bodies (IEC, BSI) and, as of 2006, is maintained by the ITU. It became widely used in Europe, especially in broadcasting, and was adopted by Dolby Laboratories who realised its superior validity for their purposes when measuring noise on film soundtracks and compact cassette systems. Its advantages over A-weighting is less understood in the US, where the use of A-weighting still predominates.[citation needed]It is universally used by broadcasters in Britain, Europe, and former countries of the British Empire such as Australia and South Africa.Though the noise level of 16-bit audio systems (such as CD players) is commonly quoted as −96 dBFS(relative to full scale), the best 468-weighted results are in the region of −86 dBFS.[citation needed][edit] Function realisation of some common weightingsAlthough they are defined in the standards by tables with tolerance limits (to allow a variety of implementations)[citation needed] the weightingscan be described in terms of a weighting function that acts on the amplitude spectrum (not the intensity spectrum), or an offset X(f) that must be added to the unweighted sound level in dB units. Appropriate weighting functions are:[4][edit] A[edit] B[edit] CThe offsets (2.0, 0.17 and 0.06 for A, B and C, respectively) ensure the normalisation to 0 dB at 1000 Hz.[edit] DwhereThis formula is adopted from [3].[edit] Transfer function equivalentThe gain curves can be realised[5] by the following s-domain transfer functions. They are not defined in this way though, being defined by tables of values, with tolerances, in the standards documents, thus allowing different realisations:[citation needed][edit] Ak A≈ 7.39705×109[edit] Bk B≈ 5.99185×109[edit] Ck C≈ 5.91797×109[edit] Dk D≈ 91104.32The k values are constants which are used to normalize the function to a gain of 1 (0 dB). The values listed above normalize the functions to 0 dB at 1 kHz, as they are typically used. (This normalization is shown in the image.)。

halcon 傅里叶变换进行空域和频段的转换

傅里叶变换及其反变换实现了图像在空间域和频域中的相互转换。

在图像处理中，傅里叶变换被广泛应用于图像增强、图像去噪、图像压缩等领域。

傅里叶变换的基本原理是将图像从空间域转换到频域。

在空间域中，图像表示为像素的强度和位置，而在频域中，图像表示为不同频率分量的幅度和相位。

通过傅里叶变换，可以将图像从空间域中的实部和虚部转换为频域中的正弦和余弦函数。

具体来说，傅里叶变换将图像的每个像素表示为一个复数，该复数包含实部和虚部。

通过将每个像素的实部和虚部乘以不同的权重，然后将它们相加，可以得到该像素在频域中的值。

这个值对应于特定频率下的幅度或相位。

在频域中，每个频率分量都对应于空间域中的一个方向。

例如，水平方向对应于0频率分量，垂直方向对应于π/2频率分量，对角线方向对应于π频率分量。

因此，在频域中处理图像可以更容易地突出某些特征，例如边缘和纹理。

傅里叶变换的逆变换是将频域中的幅度和相位转换回空间域中的像素值。

通过将每个频率分量的幅度和相位乘以不同的权重，然后将它们相加，可以得到空间域中的像素值。

这个值对应于原始图像中
的一个像素。

总之，傅里叶变换是一种重要的图像处理工具，可以将图像从空间域转换到频域，也可以将图像从频域转换回空间域。

这种转换有助于进行图像增强、去噪、压缩等操作。

octave 小波变换

octave 小波变换Octave小波变换小波变换是一种非常重要的信号分析方法，它可以将信号分解成不同频率的子信号，并可以在时间和频率上提供更好的局部信息。

在信号处理和图像处理领域，小波变换被广泛应用于信号去噪、特征提取、边缘检测等任务中。

Octave是一种开源的科学计算软件，可以用于数值计算、统计分析、信号处理和图像处理等领域。

在Octave中，我们可以使用小波变换函数进行信号和图像的分析和处理。

小波变换的基本原理是使用一组小波基函数对信号进行分解。

这些小波基函数是由母小波函数经过平移和缩放得到的。

在Octave中，我们可以使用`cwt`函数来进行连续小波变换，使用`dwt`函数来进行离散小波变换。

在连续小波变换中，我们需要选择合适的小波基函数和尺度参数。

小波基函数的选择通常根据待处理信号的特性来确定，常用的小波基函数有Morlet小波、Haar小波、Daubechies小波等。

尺度参数决定了小波基函数的频率范围，可以通过改变尺度参数来观察不同频率范围的信号成分。

在离散小波变换中，我们将信号分解为不同尺度和频率的子信号。

分解过程是通过将信号进行滤波和下采样来实现的。

通过多级的分解，我们可以得到信号的不同频率成分。

离散小波变换可以通过多级的低通滤波和高通滤波来实现，常用的小波基函数有Haar小波、Daubechies小波、Symlet小波等。

Octave中提供了丰富的小波变换函数和工具箱，例如`wavelet`工具箱和`signal`工具箱。

这些工具箱提供了各种小波基函数的定义和小波变换的实现，可以方便地进行信号和图像的小波变换分析。

在实际应用中，小波变换可以用于信号去噪。

由于小波变换具有良好的时频局部性，它可以更好地捕捉到信号的局部特征。

通过对信号进行小波变换，可以将噪声和信号的局部特征分开，从而实现信号的去噪处理。

小波变换还可以用于图像处理中的边缘检测和特征提取。

由于小波变换可以提供更好的时频局部信息，因此在图像中可以更准确地检测到边缘和纹理特征。

octave用法

octave用法Octave 是一种开源的数值计算软件，类似于商业软件MATLAB。

它提供了一个交互式环境，用于执行数值计算、算法开发和数据分析。

Octave 的用法非常广泛，它可以用于处理各种数学问题，包括线性代数、数值积分、微分方程、优化等。

它还可以用于数据可视化、信号处理、图像处理等领域。

下面我将介绍Octave 的一些常用用法。

1. 基本操作：- 变量定义和赋值：可以使用等号（=）来定义和赋值变量，例如a = 1。

- 输出：可以使用disp() 函数来输出变量的值，例如disp(a)。

- 注释：可以使用百分号（%）来添加注释，例如% 这是一个注释。

- 清除命令窗口：可以使用clc 命令来清除命令窗口中的内容。

- 帮助文档：可以使用help 命令来获取函数的帮助文档，例如help sin。

2. 数值计算：- 基本运算：支持常见的数学运算符，如加法（+）、减法（-）、乘法（*）、除法（/）和幂运算（^）。

- 矩阵操作：可以使用矩阵来表示向量和矩阵，并进行相应的运算。

例如，可以使用[1, 2, 3] 表示一个行向量，使用[1; 2; 3] 表示一个列向量。

- 线性代数：支持矩阵的转置、逆矩阵、特征值和特征向量计算等线性代数运算。

例如，可以使用inv(A) 来计算矩阵A 的逆矩阵。

- 数值积分：可以使用quad 函数来进行数值积分。

例如，可以使用quad(@(x) x^2, 0, 1) 来计算函数x^2 在区间[0, 1] 上的积分值。

- 微分方程：可以使用ode45 函数来求解常微分方程。

例如，可以使用ode45(@(t, y) t*y, [0, 1], 1) 来求解方程dy/dt = t*y，其中初始条件y(0) = 1。

- 优化：可以使用fminunc 函数来进行数值优化。

例如，可以使用fminunc(@(x) x^2, 0) 来找到使函数x^2 最小化的变量x 的值。

3. 数据处理：- 数据导入和导出：可以使用load 和save 函数来导入和导出数据。

octave的运用

octave的运用Octave是一种开源的数学软件，它被广泛应用于科学计算、数据分析和数据可视化等领域。

本文将介绍Octave的基本特点、常用功能和应用场景。

Octave是一种类似于Matlab的软件，它提供了丰富的数学函数和工具箱，可以进行矩阵运算、绘图、数据分析和模拟等操作。

与Matlab相比，Octave的一个主要优势是它是开源的，可以免费使用，而Matlab则需要购买许可证。

Octave的语法与Matlab非常相似，用户可以使用命令行界面或脚本文件进行操作。

通过Octave，用户可以进行各种数学计算，如线性代数、数值计算、统计分析和优化等。

同时，Octave也支持绘制各种类型的图表，如曲线图、散点图和柱状图等，帮助用户更直观地理解数据。

Octave的一个重要特点是它支持矩阵运算。

用户可以使用Octave 进行矩阵的加减乘除、转置、求逆和特征值分解等运算。

这使得Octave在科学计算和数据分析领域具有重要的应用价值。

例如，在机器学习中，矩阵运算常常用于处理大量的数据和参数，Octave的高效矩阵计算功能可以大大提高计算效率。

除了矩阵运算，Octave还提供了丰富的统计分析函数和工具。

用户可以使用Octave进行数据的描述性统计、假设检验、回归分析和聚类分析等操作。

这些功能对于数据科学家和统计学家来说是非常重要的，可以帮助他们从数据中提取有价值的信息和模式。

在数据可视化方面，Octave也提供了强大的绘图功能。

用户可以使用Octave绘制各种类型的图表，如线图、散点图、柱状图和饼图等。

通过这些图表，用户可以更直观地展示数据的分布、趋势和关系，帮助他们做出更准确的决策。

除了基本功能，Octave还支持各种扩展包和工具箱，用户可以根据自己的需求选择安装和使用。

这些扩展包可以提供更多的数学函数和工具，满足用户对特定领域的需求。

例如，Octave提供了信号处理工具箱、图像处理工具箱和控制系统工具箱等，为用户在这些领域的研究和应用提供了便利。

工业企业厂界环境噪声排放标准

工业企业厂界环境噪声排放标准《工业企业厂界环境噪声排放标准》，是行业行政法规，即国家环境保护部2008年第44号文件。

为贯彻《中华人民共和国环境保护法》和《中华人民共和国环境噪声污染防治法》，防治环境噪声污染，保护和改善生活环境，保障人体健康，促进经济和社会可持续发展，现批准《工业企业厂界环境噪声排放标准》和《社会生活环境噪声排放标准》两项标准为国家环境噪声排放标准，并由国家环境保护部与国家质量监督检验检疫总局联合发布。

目录1概述2环境保护部公告3环境噪声排放标准1.概述编辑《工业企业厂界环境噪声排放标准》GB12348-2008，是行业行政法规，是为防治环境噪声污染，保护和改善生活环境，保障人体健康，促进经济和社会可持续发展，而制订的环境噪声排放标准，是由国家环境保护部与国家质量监督检验检疫总局联合发布的。

2.环境保护部公告编辑(2008年第44号)关于发布《工业企业厂界环境噪声排放标准》、《社会生活环境噪声排放标准》两项国家污染物排放标准的公告发布部门:发布日期:2008年08月19日实施日期:2008年10月01日 (中央法规)为贯彻《中华人民共和国环境保护法》和《中华人民共和国环境噪声污染防治法》，防治环境噪声污染，保护和改善生活环境，保障人体健康，促进经济和社会可持续发展，现批准《工业企业厂界环境噪声排放标准》和《社会生活环境噪声排放标准》两项标准为国家环境噪声排放标准，并由我部与国家质量监督检验检疫总局联合发布。

3.环境噪声排放标准编辑为贯彻《中华人民共和国环境保护法》和《中华人民共和国环境噪声污染防治法》，防治工业企业噪声污染，改善声环境质量，制定本标准。

本标准是对GB 12348-90《工业企业厂界噪声标准》和GB 12349-90《工业企业厂界噪声测量方法》的第一次修订。

与原标准相比主要修订内容如下: ——将《工业企业厂界噪声标准》(GB12348-90)和《工业企业厂界噪声测量方法》(GB12349-90)合并为一个标准，名称改为《工业企业厂界环境噪声排放标准》;——修改了标准的适用范围、背景值修正表;of the contract must be "/"; Seven or one copy of the contract, each contract form, contents and elements; VIII, master/slave contract and complete specification interface, contract number. 5.9 other provisions the Bank supports risk managers, accompanied by professional risk management, such as account managers handle the pre-loan investigation, contracts and other key sectors. Risk managers and other professional accompanied by risk managers, account managers are still subject tothese measures (procedures) for the fulfilment of duties, qilu Bank risk managers, risk managers, in accordance with the administrativeprocedures (trial implementation) called for duties. Risk managementrisk managers and other professional personnel involved in handling,account manager with double handle. 6. the credit policy of the Bank to support capital uses reasonable and sufficient source of repayment, parties of good credit personal loans. As described in the Bank's credit policy, marketing advice, business management and other documents. 7. process description and control business processes including personal loans loan application, acceptance and investigation, risk assessmentand review, loan approval, Contracting, loan origination, loan payments, credit administration and loan recovery and disposal after nine stages. Process input for receipt of the borrower's application, the output of the process for loan recovery. Process description and control ofspecific requirements are as follows: node operation flow control point 1. loan application 1. loan borrowers applying for the loan. Risk 1:risk Description: loan qualifications did not meet the Bank requirements. Control measures: according to the preliminary information provided by the borrower selected clients shall not be artificially reduce credit terms——补充了0 类区噪声限值、测量条件、测点位置、测点布设和测量记录;——增加了部分术语和定义、室内噪声限值、背景噪声测量、测量结果和测量结果评价的内容。