[PPT]非饱和土力学大师Fredlund的演讲英文

合集下载

非饱和土力学讲义(Fredlund在香港科大)chapter2ustmod

Soil Mechanics for Unsaturated Soils Professor Delwyn G. Fredlund•The study of a multi-phase system such as an unsaturated soil, requires an understanding of the properties of each component phase.rties of each phase of an unsaturated soil; ii.) an understanding of the interaction between air and water; iii.) establishment of useful volume-mass relations for solving engineering problems.Additional notes:Soil particles &contractile skinbehave as solidsAir & waterbehave as fluids•An unsaturated soil is commonly referred to as a 3-phase system composed of: i.) solids (soil particles); ii.) water; iii.) iii.) air.•The air-water interface (i.e., the contractile skin) warrants inclusion as an additional phase due to its unique and specific properties.•The contractile skin interacts with the soil particles in an independent manner and can significantly change the mechanical behavior of an unsaturated soil.fore can be neglected in volume-mass relationships.•The air phase might be present in an unsaturated soil either in a continuous or an occluded (bubbles) form.Additional notes:Required tounderstandthe stress stateanalysisSufficient forMapping phasechanges•Gravimetric and volumetric relationships for unsaturated soil are based on various mass and volume ratios of the component phases.•A rigorous microscopic analysis of the volume-mass properties of an unsaturated soil mass requires the inclusion of the contractile skin.•Using a macroscopic (phenomenological) approach, an unsaturated soil can be considered as a three-phase continuum system.•The most important property of the contractile skin is its ability to exert a tensile pull which is a consequence of the surface tension generated between the two pore-fluid phases (i.e., air and water).Additional notes:•Air is a mixture of several gases and varying amounts of water vapor. water vapor is present.•Air is compressible and its density requires additional considerations in terms of pressure and temperature.Additional notes:Air is a mixture of several gases that act in an independent manner•Air is considered to behave as an “ideal gas” under pressures and temperatures commonly encountered in geotechnical engineering. •The molecular mass of air , ωa , depends on the mixture of dry air and water vapor.lar mass ofwater vapor is 18.016 kg/kmol.from0.000002% to 5% (Harrison, 1965). Additional notes :Density is equal to mass per unit volume Density of air is directlyproportional to the absolute air pressure•Amounts of Nitrogen (N 2) and Oxygen (O 2) are essentially constant.•Carbon dioxide (CO 2) content in air may vary depending on the rate of consumption of fossil fuels. Additional notes:•Water is essentially a homogeneous substance the world over, except for variations due to salts and isotopes of hydrogen and oxygen (Dorsey, 1940).•Distilled water under the pressure of its saturated vapor is called pure, saturated water.•The density of water must be measured experimentally.•Variations in the density of water due to temperature differences are more significant than variations due to applied pressure.Additional notes:SURFACE TENSION •A molecule in the interior of the water experiences equal forces in all directions whilea water molecule in the contractile skin experiences an unbalanced force towards the interior of the water.actile skin.alled its surface tension,T s (i.e., units of N/m 2).•Contractile skin behaves like an elastic membrane.-water surface can be related to surface tensionand the radius of curvature by considering equilibrium.Additional notes:Surface Tension Phenomena•Surface tension is measured as the tensile force per unit length along the contractile skin (i.e., units of N/m).•Surface tension is tangential to the contractile skin surface. Its magnitude decreases as temperatures decreases.u a , that is greater than thewater pressure, u w .•The pressure difference, (u a -u w ) is referred to as matric suction.Additional notes:•Contractile skin forms a warped or saddle-shaped surface (i.e., 3-D membrane) that has different curvatures along two orthogonal (i.e., R 1and R 2for example).o C.-water interface.Additional notes:State Diagram for Water(from van Haveren and Brown, 1972) •Water phase can exist in one of three states: i.) solid state as ice; ii.) liquid state aswater; iii.) gaseous state as water vapor.•The vaporization curve is also called the vapor pressure curve of water.•The fusion curve separates the solid and liquid states.•The sublimation curve separates the solid and vapor states.•Triple point of water is achieved at a temperature of 0o C and a pressure of 0.61 kPa.Additional notes:•The vaporization curve represents an equilibrium condition between the liquid and vapor states of water.•At equilibrium, evaporation and condensation processes occur simultaneously at the same rate.•Rate of condensation depends on the water vapor pressure it reaches at itssaturation value on the vaporization value.•Evaporation rate depends only on temperature.•Water vapor is mixed with air in the atmosphere, and has no effect on thebehavior of the water vapor.•Water vapor may not be in equilibrium with adjacent water. Water vapor in air can be under-saturated, saturated or super-saturated depending on the partialpressure of water vapor.•The degree of saturation with respect to water vapor is referred to as relative humidity, RH.Additional notes:•Water molecules form a lattice structure with openings referred to as a “cage” that can be occupied by gas.•Air dissolves water and fills the “cages” which comprise approximately 2% by total volume.Additional notes:•The volumetric coefficient of solubility, h,varies slightly with temperature.•The rate at which mass is transferred across a unit area is equal to the product of the coefficient of diffusion, D,and the concentration gradient.•In the diffusion of air through water, the concentration difference is equal to the difference in density between the free air and the dissolved air in the water.•The pressure difference between the free air and the air dissolved in the water becomes the driving potential for the free air to diffuse into the water.•The coefficient of diffusion for air through the pore-water in a soil appears to differ by several orders of magnitude from that for air through free water.Additional notes:•Volume of air dissolved in water is essentially independent of air or water pressures.•Absolute pressure of the dissolved air is equal to the absolute pressure of the free air under equilibrium conditions.•Volume of air dissolved in water is constant for different pressures.•Ratio between the mass of each gas that can be dissolved in a liquid and the mass of the liquid is called the Coefficient of Solubility , H.•Coefficients of Solubility are referenced to a standard pressure of 101.3 kPa.•Ratio of the volume of dissolved gas, V d ,in a liquid to the volume of the liquid is the Volumetric Coefficient of Solubility , h.Additional notes :•A cylinder with a piston and porous stone analogy is useful in visualizing and analyzing the behavior of air-water mixtures.•The “porous stone” has pores equaling 2% of its volume. The “porto simulate the behavior of water.•An initial pressure is applied equally to the free air and the “•An imaginary valve is placed at the boundary between the free air and the “porous stone”.•When the piston is loaded, it compresses the free air above theporous stone inaccordance with Henry’s law.•Henry’s law states that the mass of gas dissolved in a fixed quantity of liquid, at constant temperature, is directly proportional to the absolute pressure of the gas above the solution (Sisler et al., 1953).•After several increases of the applied loading, all free air will have moved into the “porous stone”, and any additional applied load will be carriedAdditional notes:•The water lattice is relatively rigid and stable (Dorsey, 1940) and the density of water changes little as a consequence of the presence of the dissolved air.•Dissolved air has no measurable effect to the density of water at a temperature of about 22o C.Additional notes:•An unsaturated soil has air and water pressures at different magnitudes.•The mass of air going into or coming out of the water is time dependent.•The amount of gas dissolved in water is referred to as its Solubility .•The rate of solution of a gas is referred to as its Diffusivity .Additional notes:•The volume-mass relations of the soil particles, water, and air phases are useful properties in engineering practice, particularly for an unsaturated soil.•Both mechanical and hydraulic behaviors of an unsaturated soil mass are highly dependent on the relative proportion of the three component phases (i.e., solid, water and air).•Soil properties such as degree of saturation, porosity and void ratio express volumetric proportions involving the three phases of an unsaturated soil mass.•The above volumetric properties associated with the density of each phase allows the definition of all the index properties commonly utilized in engineering practice to characterize the state of a soil mass.Additional notes:•Each phase of an unsaturated can be expresses as a percentage of the totalvolume by using the definition of porosity.•The volume of the contractile skin can be assumed to be negligible.•The porosity with respect to the water phase, n w ,is equivalent to the volumetric water content, θw .•A soil mass might change its total volume in response to changes in the stress state applied. However, the volume of solid particles is constant.•Void ratio of a soil mass expresses the ratio of the void volume to the volume of the solid particles.Additional notes:•The degree of saturation expresses the percentage of void space filled by the liquid phase (i.e., water).dry (i.e., S = 0%),saturated (i.e., S = 100%) or unsaturated ( 0% < S < 100%).•The transition zone between a continuous air phase and occluded air bubbles occurs when the degree of saturation is between approximately 80-90%.•The water content can also be measured either in a gravimetric manner (i.e., ratio of water to solids mass) or in a volumetric manner (i.e., ratio of water volume to total volume).Additional notes:•Two commonly used soil density definitions are the total density and the dry density. Total density is called the bulk density.•Dry density of a soil mass is dependent upon the mineralogy of the solidphase, in terms of specific gravity,G s , and the porosity of the soil structure.•The saturated density of the soil is the total density of the soil for the casewhere the voids are filled with water (i.e., S = 100%).•The buoyant density of a soil is the difference between the saturated density of the soil and the density of water.•A “basic volume-mass relationship” for a soil mass can be derived by using the idealized diagram where each soil phase is defined by its volume and its mass.Additional notes:•Volume-mass relationships in engineering practice neglect the mass of airphase, but consider the volume of the air phase.•The expression (Se = wG s )is the basic volume-mass relationshipcommonly used in engineering practice and it represents the continuityequation for a soil mass.•Additional notes:•Density, ρis the ratio of mass to volume. Each soil phase has its own density.•Specific gravity of the soil particles, Gs ,is defined as the dimensionless ratio between thedensity of the soil particles and the density of water at a temperature of 4o C underatmospheric pressure conditions (i..e, 101.3 kPa).•Density of water at 4o C and at 101.3 kPa is 1000 kg/m 3.•Specific volume, v o ,is the inverse of density (i.e., ratio of volume to mass).•Unit weight, γis the product of density, ρ, and gravitational acceleration, g,(i.e., 9.81 m/s 2).Additional notes:•In engineering practice, the total density is often increased by imposing a reduction in the void volume of a soil mass.•Volume of pore-water in a soil mass is highly dependent uponenvironmental conditions and therefore is often a variable to be considered.•The dry density is independent of the volume of the pore-water and is often used in semi-empirical relationships for the soil.Additional notes:•The dry density curve corresponding to a degree of saturation of 100 % is called the “zero air voids”, ZAV, curve.•The dry density curves curves for different degrees of saturation should be presented in connection with soil compaction data.•Compaction is a mechanical process used to increase the dry density of soils (i.e., densification).Additional notes:-Start of process:•The basic volume-mass relationship (i.e., Se = w Gs ) applies to anycombination of S, e and w , and therefore it can be used to obtain changes in any of these properties when the other two properties are defined.•The basic volume-mass relationship can therefore be used to deriveequations associating changes of S, e and w .Additional notes:•Soil mixtures can occur in various forms in nature.•Mixture of soil particles and air constitutes a dry soil while a mixture of soil particles and water constitutes a saturated soil.•Unsaturated soils lie between dry and saturated soil conditions and constitute a mixture of soil particles, air and water in differing percentages.•The density of a soil mixture can also be formulated for situations where there is a change in the volume of air due to compression under undrained conditions. •The analogy of the piston-porous stone is a useful tool to derive the formulation of the density of the soil by considering the air compression.•In the above formulation, Boyle’s law is applied to the free and dissolved air under isothermal conditions.Additional notes:•Under isothermal conditions, the ratio of the density to the absolute pressure of the air phase (i.e., free and dissolved air) in a soil mixture is constant.•The final density of water is computed as the ratio of the total mass of water and dissolved air to the total volume of water and dissolved air under final equilibrium conditions.Additional notes:•The conservation of mass is herein applied to a system (i.e., soil mixture) under isothermal conditions, therefore, all the previous equations can be applied. •The total density of the soil mixture is therefore a function of the constant densities and volumes of water and solid phases as well as of the variable density and volume of the air phase.•The total density formulation can be applied to the definition of particular cases where one of the three phases (i.e., solid, water and air) in a soil mixture is not present.Additional notes:•The first equation above is a general mixture equation for density after the soil has been subjected to a change in air pressure.•The volume of dissolved air , V d ,is related to the volume of water, Vw ,by thevolumetric coefficient of solubility, h (i.e., V d = hV w ).•The equation incorporates the effect of air going into solution due to the change in the air pressure.•Where air does not have time to dissolve in water, the volumetric coefficient of solubility, h,might be set to zero.•The air-water mixture always has a density between that of air and water.•The density of an air-water mixture can be obtained from the general equation is obtained by setting the volume of the soil particles, Vs ,to zero, and the initial voidratio, e i ,becomes infinity.Additional notes:。

不饱和土力学英文课程课件CHAP 6_Mech of Unsat Soils_Vanapalli_2016

Chapter 6SHEAR STRENGTH OF UNSATURATED SOILS •Shear strength of a soil is required for addressing numerous problems such as design of foundations, retaining walls, slopes and pavements.•Equation for interpreting the shear strength of soils:c’ = effective cohesionσ = total normal stressu w= pore-water pressureφ’ = angle of internal friction•φ’ for gravels > sands > silts >clays (Recall the φ’ values)•Saturated shear strength of soils is related to one stress state variable;namely, effective stress, (σ – u w).•Shear strength parameters are commonly determined using triaxial shear tests, direct shear tests, or ring shear tests.Figure. Triaxial shear test equipment: a versatile equipment in whichdifferent types of drainage conditions can be introduced and tested •The shear strength of a saturated soil is commonly described extending the Mohr-Coulomb failure criterion from triaxial shear tests and the effective stress concept as given below:Figure. Mohr-Coulomb shear strength failure envelope for a saturated soil using triaxial shear test results (modified after Terzaghi, 1943N σ′=As=Figure: Shear strength envelope from direct shear strength test results Triaxial shear test (Versatile test)Figure: Comparison of CU and CD shear strength test results of a cohesive soil using identical soil samples.Figure: Reasons associated with the changes in shear strength from CU and CD tests.Figure: Comparison of CD and UU shear strength test results of a cohesive soil using identical soil samplesFigure: Comparison of CD and UU shear strength test results of a cohesive soil using identical soil samplesFigure: Comparison of shear strength envelops for UU, CU and CD tests•Unconfined compression test: Special case of triaxial shear test with no confining pressure (i.e., σ3 = 0).•It is convenient to measure the undrained shear strength of cohesive soils using unconfined compression tests (this value is typically used in the design of foundations).Figure: Unconfined compression test•Practical engineering applications of drained and undrained loading conditions.Drained Loading(i) Long term stability (ii) Steady state seepage (iii) Shallow foundations (iv) Slow rates of construction (v) Seepage control during constructionUndrained Loading(i) During construction (Rapid): Embankments, Cuttings(ii) Sudden draw down: Dam reservoirs, Floods(iii) No seepage control: Embankments, Cuttings(iv) Earthquake loading•Soils that are in a state of unsaturated condition have to be interpreted in terms of two stress state variables; namely, net normal stress, (σ– u a)and matric suction, (u a – u w).•The conventional triaxial and direct shear testing equipment used for testing saturated soils must be modified to test unsaturated soils.•Similar to saturated soils, shear strength testing of unsaturated soils involves two stages (i.e., first stage is loading and the second stage is shearing).•The pore-air pressure, u a and the pore-water pressure, u w, are independently maintained both during the process of loading and shearing using axis-translation technique.•The axis-translation technique is used to translate the negative pore-water pressure, u w to a pressure that can be measured without cavitation of the water.• A high air-entry disk with an air-entry value greater than the matric suction, (u a – u w) in the soil is used in order to prevent the passage of pore-air into the measuring system.•It is very important to use “identical” specimens for determining the shear strength of soil.•In the case of compacted soils, the soil specimens need to be compacted at the same initial water and dry density conditions in order to qualify as “identical” specimens.Figure: Differences in soil specimens due to the influence of soil structure associated with changes in water content.•Specimens compacted at the same water content but at different dry densities, or vice versa, cannot be considered as “identical” soils, even though their classification properties are the same.• Soils with differing density and water content conditions can yield different shear strength parameters and should be considered as different soils.• The shear strength is performed by loading a soil specimen with increasing applied loads such that a failure load is determined from the stress versus strain relationships.Figure: Strain concept used in the triaxial test• Various types of failure criteria are defined in the literature from stress versus strain relationships.Figure: Stress versus strain curves for consolidated drained tests and undrained triaxial tests• In certain cases failure load is not well defined (as shown below). An arbitrary strain rate (e.g., 12%) is selected to represent failure conditions.Figure: Strain limit as a failure condition• A series of tests should be conducted such that the shear strength is determined over a range of suction values using “identical” specimens with the same net normal stress, (σ – u a ).Figure: Shear strength envelope for an unsaturated soilFredlund et al. (1978) shear strength equation for unsaturated soils.()()b f w a fa f ff tan u u 'tan u 'c φφστ−+−+=where: τff = shear stress on the failure plane at failure,c’ = intercept of the “extended” Mohr-Coulomb failure envelope on the shear stress axis where the net normal stress and the matric suction at failure are equal to zero; it is also referred to as “effective cohesion”,(σf – u a )f= net normal stress state on the failure plane at failure,Special Design Considerations•Conventional equipment used for the measurement of the shear strength of saturated soils requires modifications when testing the shear strength of unsaturated soils.•Modifications should accommodate independent measurement (or control) of the pore-air pressure, u a, and the pore-water pressure, u w.•The axis translation technique forms the basis for these modifications such that laboratory testing of unsaturated soils with high matric suctions can be undertaken.Figure: Illustrates the application of axis-translation technique to a soil specimen with matric suction of 100 kPa.-The pore-water pressure, u w, is measured below a saturated air-entry disk that has an air-entry value of 2 bars (202.6 kPa).-Air-pressure, u a, of 180 kPa is applied directly to the specimen in order to raise the pore-air (and pore-water) pressures.-The pore-air pressure raised to 180 kPa increases the pore-water pressure by an equal amount.-Thus, the pore-water, u w,will be at a positive value of 80 kPa and will be at a positive value of 80 kPa.•Pore-air diffuses through water if the axis-translation technique is extended for a long time.•D iffused A ir V olume I ndicator (DAVI) can be used in conjunction with the measuring system in order to flush diffused air from below the high air-entry disk.•Procedure for saturating the high-air entry disk- A head of water is to be created on the disk in the triaxial or direct shear testing equipment and allow water to flow for a period of one hour using air-pressure 5or 6 times the value of the air-entry value of the stone.- The air-bubbles collected during the process from below the disk are flushed out.• A highly flexible pressure measuring system has slow response to pore-water pressures. Recommended to use a rigid measuring system.•The specimens that are used for testing the shear strength of unsaturated soils are either undisturbed or compacted.•These specimens are saturated prior to conducting the shear strength test. (i.e., typically drying path is followed).•Saturation in triaxial shear equipment is achieved by incrementally increasing the pore-water pressure, u w. At the same time, the confining pressure, σ3, is increased in order to maintain a constant effective stress, (σ3 – u w).•As a result, the pore-air pressure increase and the pore-air volume decreases by compression and dissolution into the pore-water.Triaxial Shear Tests for Unsaturated Soils•Various types of triaxial test methods are defined on the basis of drainage conditions during the application of confining pressure and during the application of shear stress.•The test methods are similar to saturated soil testing (i.e., CD, CU, UU etc..)•In most of these tests, the net total confining pressure, (σ3 – u a), generally remains constant during shear.•The axial stress is continuously increased until a failure condition is reached.•The shear strength is interpreted in terms of two stress state variables, (σ – u a) and (u a – u w).•Effective stress analysis approach can be used if the stresses at failure conditions are known.•The shear strength has to be interpreted in terms of total stresses at failure, if the pore pressures are not measured or controlled.•The total stress approach should be applied for undrained loading conditions. •Example of undrained loading conditions for unsaturated soils: Rapid loading of a fine-grained soil.Pore-air Pressure Control System•Figure above shows arrangement for pore-air pressure control using triaxial equipment.• A 3.2 mm thick, coarse corundum disk is placed between the soil specimen and the loading cap.•The disk is connected to the pore-air pressure control through a hole drilled in the loading cap, connected to a small-bore polyethylene tube.•The measurement of pore-air pressure can be achieved using a small pressure transducer.•Pore-air pressure is difficult to measure because of the ability of air to diffuse through rubber membranes, water, polythene tubing, and other materials.Table 1: Various triaxial testing conditions with necessary measurementsFigure: Various stress conditions during a consolidated drained triaxial shear test.Figure. Triaxial base plate for unsaturated soil testing. (a) Plan view of the base plate with its outlet ports; (b) cross-section of a base plate with a high air entry disk (from Fredlund and Rahardjo, 1993).Valve A: To control the pore-water pressure and to measure the water change during a drained shear test.Valve B: Used for pore-water measurement, flushing system, and diffused air volume measurement.Valve C: Pore-air pressure control or measurement.Valve D: Cell pressure control and measurement.The grooves inside the water compartment serve as water channels for flushing airbubbles that might be trapped or have accumulated as a result of air diffusion.Figure: Layout of the plumbing for modified triaxial shear testing.•Unsaturated soil testing requires several procedural checks.•The high air-entry disk should be saturated.•Attempts should be made to thoroughly flush water through the compartment in the base plate and all connecting lines, to ensure the expulsion of air bubbles.•The volume change measuring devices, including the diffused air volume indicator, should be initialized.•Briefly discuss the step-by-step procedure of conducting a CD triaxial test fordetermining the shear strength parameters for an unsaturated soil?Various Triaxial Tests for Unsaturated SoilsUndrained Tests•The procedure for performing an undrained test on an unsaturated soil specimen using the triaxial test is similar to the procedure used for performing an undrained test on a saturated soil specimen.•The unsaturated soil specimen is tested at its initial water content or matric suction. •The initial matric in the specimen is not relaxed or changed prior to commencing the test.•Both confined and unconfined compression triaxial tests can be conducted and the shear strength contribution due to suction can be interpreted in terms of total stresses.Unconfined Compression Test•The unconfined compression is a special case of undrained test and usually performed in a simple loading frame without the application of any confining pressure (i.e., σ3 = 0).Figure: Unconfined compression test apparatus•The compressive load is applied quickly in order maintain undrained conditions (the specimen is sheared in 10-15 minutes).•Theoretically, this would mean that there would be significant changes in both the pore-air pressure and the pore-water pressure in the soil specimen (i.e., a valid assumption for compacted fine-grained soils).•The pore-air and pore-water pressures are not commonly measured during compression.•The deviator stress, (σ1– σ3)is equal to the major principal stress, σ1, since the confining pressure, σ3 is equal to zero.•The deviator stress at failure is referred to as the unconfined compressive strength, q u.•Unconfined compression test is a special case of triaxial shear tests with σ3 = 0.Confined Compression Test•The undrained shear strength for the unsaturated soil increases as the confining pressure increases.Figure: Results of confined compression tests on four “identical” soil specimens (i.e., having equal density, water content and matric suction).•Note that the four “identical” soil specimens are brought to different initial states of stress, due to the changes in pore pressures under undrained loading conditions.Figure. 1. Development of pore-pressures due to undrained loading conditions •The changes in the pore-air pressures and pore-water pressures can be determined using the theory of undrained pore pressure parameters (i.e., B a and B w parameters) or can be measured at the end of the test (Fredlund and Rahardjo, 1993).•The shear increases with an increasing confining pressure. At the same time, matricsuction in the soil specimen decreases with an increase in degree of saturation accompanied by a reduction in the volume.•The diameter of the Mohr’s circles increase with an increase in confining pressure. •The envelope defines a curved relationship between the shear strength and totalnormal stress for unsaturated sols tested under undrained conditions.•Once the soil becomes saturated under the application of confining pressure a horizontal envelope develops with respect to the shear strength axis.•Once the soil is saturated the shear strength behavior is in accordance with the φu =0 concept.In a triaxial test the total stress increment d σ2 equals d σ3. The major principal stress increment of total stress, d σ1, is applied axially. The development of pore pressures in the undrained triaxial test are influenced both by the total stress increment, d σ3 and from the change in the deviator stress, d(σ1 - σ3).The changes in pore pressures are given by:()du B d D d a a a =+−σσσ313[5] ()du B d D d w w w =+−σσσ313[6]Equations 5 and 6 can be expressed in a different form as:{})31(d a A 3d a B a du σσσ−+=[7]{})31(d w A 3d w B w du σσσ−+=[8]where:a D a A a B = [9] w D w A w B =[10]Shear strength can be related to the total stresses when the pore pressures at failure are unknown. In undrained triaxial tests, the specimens when subjected to increasing loading, undergo changes in pore-air and pore-water pressures which results in changes of matric suction of the soil. Such a situation in laboratory testing is demonstrated using Figure 2. Four "identical" soil specimens subjected to different confining pressures during an undrained test are shown. The pore pressures in these soil specimens increase with the increase in confining pressure. The shear strength also increases with the increasing confining pressure. The matric suction in the soil decreases with the increase in degree of saturation accompanied by a reduction in the volume. The four identical soil specimens are brought to different initial states of stress, due to the changes in pore pressures under undrained loading conditions.Direct Shear Test for Unsaturated SoilsFigure. Modified direct shear equipment for testing an unsaturated soil specimen. (a) Plan view of the pressure chamber for a direct shear box; (b) cross-sectional view A-A of a direct shear box and pressure chamber (from Gan and Fredlund 1988).Figure: Cross-sectional view of the modified direct shear equipment •Direct shear tests are commonly conducted in consolidated drained conditions. •Test procedure:- A saturated soil specimen is placed in the direct shear box and consolidated under a vertical stress, σ.-During the process of consolidation, pore-air and pore-water pressure must be controlled at selected pressures.- A known value of matric suction is imposed on the specimen using axis-translation technique.-At the end of consolidation, the soil specimen has a net vertical stress of (σ – u a) and a matric suction of (u a – u w).-During shear, the pore-air and pore-water pressure are controlled at constant.-Shearing is achieved by horizontally displacing the top half of the direct shear box relative to the bottom half.-Shear stress is increased until the specimen fails.Figure. Stress conditions during a consolidated drained direct shear test. Matric suction, (kPa)(u - u )a w S h e a r s t r e s s , (k P a )10020030040050055050150250350450050100150200250300U p p e r e n v e l o p e B e s t f i t L o w e r e n v e l o p e c = 10 kPa= 25.5( - u )ff af tan = 34.4 kPa average = 72.2 kPa( - u )ff af Initialvoid ratio, e 0(kPa)0.7771.30.5371.60.6972.80.5172.60.5472.7( - u )ff afFigure. Variation of shear strength with respect to suction from consolidated drained direct shear test.The failure envelope can be obtained for the results of direct shear tests without constructing Mohr’s circles (see figure above). The direct shear test is useful for testing unsaturated soils due to the short drainage path in the specimen. Considerably short period of time is required for conducting direct shear test in comparison to triaxial shear test.Shear strength of unsaturated soils and its applications ingeotechnical engineering practiceS.K. VanapalliCivil Engineering Department, University of Ottawa, Ontario, CanadaABSTRACT: Several investigators over the last 50 years have contributed to ourpresent understanding of the shear strength of unsaturated soils (SSUS). Manyadvances have been made during this period with respect to the measurement,interpretation and prediction/estimation of the SSUS. The focus during the last fifteenyears has been directed more towards the development of simple techniques forpredicting or estimating the SSUS because experimental procedures are timeconsuming, need elaborate equipment, and require highly trained personnel. Shearstrength is a key engineering property required in the design of several geotechnicalstructures such as the shallow and deep foundations, earth structures includingexcavations and the stability of slopes. However, limited studies are reported in theliterature with respect to the applications of the SSUS in practice. This paper providesa brief background of the SSUS and details of how our present understanding can beextended for several geotechnical applications.•Read the above Keynote paper published by Sai Vanapalli in Asia-Pacific International Conference, Australia, 2009.•PowerPoint presentation and Paper available on the class website.。

非饱和土力学03-吸力与SWCCPPT

Suction
孔隙水与土颗粒间相互作用而产生的吸力中的各个部分
毛细作用吸附作用
因溶质溶解作用而产生的吸力
渗透作用
17
1. 吸力的概念
基质吸力的探讨 Matric suction
➢ 源自毛细与短程吸附综合作用的吸力通常定义为基质吸力，它具有与压力一样的单位。
➢ 基质，是指细小的土颗粒。基质吸力可以看作是土基质对水的吸持潜能。
力学角度对土的吸力及其组成下来定义。这些定义已在岩土工程学中被广泛接受。
➢ 土中吸力反映土中水的自由能状态
4
1. 吸力的概念
自由能
➢ 什么是自由能？ free energy
在热力学当中，自由能指的是在某一个热力学过程中，
系统减少的内能中可以转化为对外做功的部分，它衡量的是：在一个特定的热力学过程中，系统可对外输出的“有用能量”。
9
1. 吸力的概念
孔隙水总势能
p T g o c ad
➢ 忽略温度、重力与惯性的影响，促使土体孔隙水势能降低的主要因素有：渗透作用——孔隙水中溶质溶解的结果毛细作用——水-气交界面曲率以及负孔隙水压力吸附作用——固-液（即土中孔隙水）交界面附近的电场与范德华力场作用而产生
1. 吸力的概念
基质吸力的探讨
➢ 基质吸力中的毛细部分和粘吸部分在概念上的区分是明显的，但难以通过试验手段加以区分
➢ 基质吸力中的各个部分对非饱和土行为影响的机理并不相同。对于低塑性的或较高含水量下的土体，基质吸力中毛细部分占支配地位；然而对于高塑性的粘土或较低含水量下的土体，基质吸力中粘吸部分占支配地位。
c (ua uw )
➢ 通常，基质吸力中的毛细部分可表示为：

fredlund非饱和土基质吸力的测定方法

了解不饱和土壤的行为就像潜入我们脚下的新世界揭开它的秘密的关键一步是用弗雷德伦德的方法找出母体的吸积这种方法类似于用宝图寻找土壤的含水量与其吸积潜力之间的隐藏关系。

母吸吸基本上是土壤的表达方式 "嘿，我可以吸水出自己！" 了解这一点对了解土壤在不同条件下的存留方式至关重要。

把母性吸吸视为不饱和土壤的超能力，准备被释放出来并揭示其机械秘密！
为了测量土壤的干燥程度，我们使用一种叫做抗辐射计的奇特工具。

这就像一个探测器我们坚持在地面我们感兴趣的水平。

探测器有一个小杯子触碰土壤中的水。

当土壤真的干燥时，它把水吸进杯子中，我们可以测量压力以判断土壤的干燥程度。

这有点像用高科技稻草看土壤有多渴！
必须强调，Fredlund在不饱和土壤中测量母体吸附的方法要求仔细注意测距仪的选择和安装，以及准确校准和明智地解释数据。

遵守土壤样品制备和测试程序的标准规程对于保持母体吸附测量的精确性和可靠性至关重要。

只有通过严格运用既定准则和程序，才能确保数据的完整性，并维持调查结果的有效性。

非饱和土抗剪强度公式分类及总结

非饱和土抗剪强度公式分类及总结张常光;赵均海;朱倩【摘要】The shear strength formulae for unsaturated soils were divided into five categories, such as using soil-water characteristic curve, mathematical fitting, piecewise functions, total stress indicators and other forms. Characteristics and deficiencies of the current research on shear strength formulae for unsaturated soils were analyzed. The results show that some differences in mechanical significance exist between shear strength formulae based on the effective stress and shear strength formulae based on the two-stress state variables. Different expressions of suction strength lead to diversities of the shear strength formulae. True triaxial tests of unsaturated soils are urgent need to be carried out and strength theory of unsaturated soils satisfying the actual engineering stress conditions is to be established in order to improve the theoretical basis of unsaturated soils and accelerate the process of engineering applications.%将众多非饱和土抗剪强度公式分为结合土-水特征曲线、数学拟合、分段函数、总应力指标及其他形式5类,分析了当前非饱和土抗剪强度公式的特点及研究不足.结果表明:有效应力抗剪强度公式和双应力状态变量抗剪强度公式的力学本质不同,吸附强度表达式的不同导致了抗剪强度公式的多样性,急需加强非饱和土真三轴试验研究,建立符合工程实际受力状况的非饱和土强度理论,完善非饱和土的理论基础,并加快非饱和土强度理论的工程实践与应用进程.【期刊名称】《建筑科学与工程学报》【年(卷),期】2012(029)002【总页数】9页(P74-82)【关键词】非饱和土;基质吸力;抗剪强度公式;真三轴试验【作者】张常光;赵均海;朱倩【作者单位】长安大学建筑工程学院,陕西西安 710061;长安大学建筑工程学院,陕西西安 710061;长安大学建筑工程学院,陕西西安 710061【正文语种】中文【中图分类】TU4320 引言非饱和土分布十分广泛，与工程实践密切联系的地表土几乎全都是非饱和土［1］。

高等土力学思考题与概念题

思考题第一章：1．对于砂土，在以下三轴排水试验中，哪些试验在量测试样体变时应考虑膜嵌入 (membrane penetration)的影响？HC, CTC, CTE, RTC, RTE, 以及平均主应力为常数的TC TE 试验。

2．对于砂土，在常规三轴固结不排水(CU)压缩试验中，围压σ3为常数，其膜嵌入 (membrane penetration)效应对于试验量侧的孔隙水压力有没有影响，为什么？对于常规三轴固结排水试验对于试验有无影响？3．对于砂土，在常规三轴固结不排水(CU)压缩试验中，围压σ3为常数，其膜嵌入 (membrane penetration)效应对于试验的不排水强度有没有影响，4．在周期荷载作用下饱和砂土的动强度τd (或σd )如何表示？定性绘出在同样围压σ3，不同初始固结比σ1/σ3下的动强度曲线。

5．在一定围压下，对小于、等于和大于临界孔隙比e cr 密度条件下的砂土试样进行固结不排水三轴试验时，破坏时的膜嵌入对于量侧的孔隙水压力有何影响？对其固结不排水强度有什么影响（无影响、偏大还是偏小）？6．在土工离心模型试验中进行固结试验，如果模型比尺为100，达到同样固结度，模型与原型相比，固结时间为多少？7．举出三种土工原位测试的方法，说明其工作原理、得到的指标和用途。

8．对于粗颗粒土料，在室内三轴试验中常用哪些方法模拟？各有什么优缺点？9．真三轴试验仪器有什么问题影响试验结果？用改制的真三轴试验仪进行试验，其应力范围有何限制？10．在饱和土三轴试验中，孔压系数A 和B 反映土的什么性质？如何提高孔压系数B ？11．在p, q 坐标、⎺σ,⎺τ坐标和在π平面坐标下画出下面几种三轴试验的应力路径（标出应力路径的斜率）。

（1） CTC （常规三轴压缩试验）（2） p ＝常数，b=0.5＝常数，真三轴试验；（3） RTE （减压的三轴伸长试验）。

其中：22)()()(213/)(31312132********σστσσσσσσσσσσσσ－＝＋＝-+-+-=++=q p)(32tan 31312σσσσσθ---=第二章1．土的刚塑性本构模型与增量弹塑性模型表现的应力应变关系曲线有何区别？2．在剑桥模型中，物态边界面上的不排水三轴试验的有效应力路径向p '--q 平面的投影是不是其屈服轨迹？为什么？3．剑桥模型是否可以反映土由于剪应力引起的体积膨胀（剪胀），清华弹塑性模型是否可以反映土由于剪应力引起的体积膨胀？二者的区别是由于什么不同？4．剑桥模型的帽子屈服面能不能反映土由于剪应力引起的体积变化？它是剪胀还是减缩？5．Duncan-Chang 模型与剑桥模型都是在常规三轴试验基础上建立的，前者通过常规三轴试验确定的(σ1-σ3)~ε1~εv 的关系推出模型参数；后者通过三轴试验建立了用p '--q 表示的模型屈服函数。

高等土力学-习题解答-李广信

第3章习题摩尔-库仑公式推导:ϕ+ϕσ+σ=σ-σcos c sin 223131 即: 231231]cos c 2sin )[()(ϕ+ϕσ+σ=σ-σ,同理有;232232]cos c 2sin )[()(ϕ+ϕσ+σ=σ-σ; 221221]cos c 2sin )[()(ϕ+ϕσ+σ=σ-σ破坏面条件:{}{}{}0]cos c 2sin )[()(]cos c 2sin )[()(]cos c 2sin )[()(221221232232231231=ϕ+ϕσ+σ=σ-σ⨯ϕ+ϕσ+σ=σ-σ⨯ϕ+ϕσ+σ=σ-σ⎪⎪⎪⎭⎪⎪⎪⎬⎫⎪⎪⎪⎩⎪⎪⎪⎨⎧+⎪⎪⎭⎪⎪⎬⎫⎪⎪⎩⎪⎪⎨⎧π-θ-θπ+θ=⎪⎭⎪⎬⎫⎪⎩⎪⎨⎧σσσ1112321I 31I 31I 31)6cos()sin()6cos(J 32 将该式代入上式得:0cos C J )3sin sin (cos sin I 3121=ϕ+ϕθ+θ-ϕ π平面上各轴的投影:在1σ轴上的投影:2S 2321321=σ-σ-σ在2σ轴上的投影:2S 2322312=σ-σ-σ在3σ轴上的投影:2S 2323213=σ-σ-σ如: 1σ=400kPa, 2σ=3σ=100kPa. 则在三个轴上的投影分别为: 141kPa, -71kPa, -71kPa.1、临界状态：是指土在常应力和常孔隙比下不断变形的状态。

临界孔隙比：表示土在这种密度状态下，受剪作用只产生剪应变而不产生体应变。

水力劈裂：由于孔隙水压力的升高，引起土体产生拉伸裂缝发生和发展的现象。

饱和松砂的流滑：饱和松砂在受静力剪切后，因体积收缩导致超孔压骤然升高，从而失去强度和流动的现象。

真强度理论：为了反映孔隙比对粘土抗剪强度及其指标的影响，将抗剪强度分为受孔隙比影响的粘聚分量与不受孔隙比影响的摩擦分量。

通过不同的固结历史，形成等孔隙比的试样，在不同的法向压力下剪切，试样破坏时的孔隙比相同，强度包线即为孔隙比相同的试样的强度包线，该强度称为在此孔隙比时的真强度。

非饱和土土力学(新)

温度
3 ua 200kPa
u a u w 100 kPa
15℃ 30℃ 45℃ 60℃

温度的影响
非饱和土土力学理论
4、非饱和土的应力应变关系及本构模型
（1）弹性模型
Fredlund和Morganstern(1976)、Fredlund(1979)提出了基于双应力变量 ( u a ) 和 (ua u w ) 的弹性应力应变关系：
强度分析：
固结变形分析：
传统（经典）土力学的局限
3、现有土工试验仪器主要是针对饱和土设计的
① 试验数据按饱和土相关理论来整理，不符合实际情况。 ② 不能测负孔隙水压力。 ③ 未考虑气相影响。
单轴压缩
三轴压缩
非饱和土土力学理论
非饱和土物质组成：固体、气体和液体
固体
液体
气体
n
Vpores Vtotal
固结本构方程
Mechanical constitutive law
q w k grad( pw w z)
d v m dp
1 dp K
pw 1 p k 2 pw wm t 3 t
3-D consolidation
传统（经典）土力学的局限
2、固结变形和强度分析中有效应力的表现形式是不一致的

Vliquid Vgas Vtotal
Sl
Vliquid Vpores

Vliquid Vliquid Vgas
饱和度
1 Sg
空隙度
非饱和土土力学理论
非饱和土为固、液、气相及收缩膜组成的四相介质
非饱和土土力学理论
存在一个新的应力状态变量：吸力

非饱和土力学讲义(Fredlund在香港科大)chapter5ustmod

Professor Lee Barbour•The modeling of unsaturated flow through soils is the most commonly used application of unsaturated soil mechanics.•Emphasis upon the environmental and geo-environmental engineering areas, in addition to classical soil mechanics problems, has resulted in the development of severalcomprehensive software computer codes.•This chapter deals with the flow of the fluid phases (i.e., air and water) through an unsaturated soil.•The analysis of fluid flow through an unsaturated soil requires the definition of the driving potential and a flow law associated to each fluid phase.•The diffusion of air through water is also discussed as a phenomenon associated to unsaturated soils.Additional notes:•The analysis of fluid flow requires a law to relate the flow rate to the driving potential through the use of appropriate coefficients.•Air in an unsaturated soil can occur either in an occluded form or a continuous form depending upon the degree of saturation of the soil.•A knowledge of the driving potentials that causes air and water to flow through an unsaturated soil is necessary for solving geotechnical engineering problems.•The movement of air through the water phase is referred to as air diffusion and its driving potential needs to be defined.•Water flow is caused by a hydraulic head gradient, where the hydraulic head consists of an elevation head plus a pressure head.•The flow of air in the continuous phase form is governed by a concentration or pressure gradient. The elevation gradient has a negligible effect.Additional notes:•What is the driving potential for the flow of water through an unsaturated soil?•Several concepts, such as water content gradient, pressure head gradient, soil suction gradients, and hydraulic head gradient have been used to explain the flow of water through an unsaturated soil.•The above figure illustrates some hypothetical cases which demonstrate that matric suction gradient is not the driving potential that causes water to flow through an unsaturated soil.•Case 1 illustrates that water can flow through an unsaturated soil from a region of low matric suction to another region at a relatively high matric suction.•Case 2 illustrates that the flow of water through an unsaturated soil can occur between regions with equal matric suctions.•Case 3 illustrates that water can flow through an unsaturated soil even when the left-hand side (i.e., departing region) is at a matric suction higher than the right-hand side (i.e., target region).•Water flow can be defined most appropriately in terms of a hydraulic head gradient. •The special case where the air pressure is zero, which is the common situation in nature, the matric suction gradient is numerically equal to the pressure gradient in the water.•The elevation head can be omitted then the matric suction gradient is equal to the hydraulic head gradient and all points in the soil mass are at the same elevation (i.e., horizontal flow).Additional notes:No suctiongradient•A point (e.g., an elemental volume) in the water phase has three primary components of energy; namely, gravitational, pressure, and velocity.•Point A has a gravitational potential energy, Eg , which is a function of the water element mass and the distance, y , relative to a datum.•When the water density, ρw , is constant, the pressure energy can be expressed as a function of the pore-water pressure, the water volume, and the water density at point A .•The flow rate of water at point A gives rise to a velocity energy that can be expressed as a function of the mass of water and the velocity of water at point A .•The total energy (i.e., mechanical energy) at point A is the summation of thegravitational, pressure, and velocity components.•Potential or hydraulic head is defined as energy per unit of weight. Therefore, the hydraulic head consists of three components; namely, the gravitational head, the pressure head and the velocity head.•The velocity head in a soil is commonly negligible in comparison to the gravitational and the pressure heads.Additional notes:•The heads used in the definition of hydraulic potential have the dimensions of length. •Hydraulic head is a measurable quantity, the gradient of which causes flow in saturated and unsaturated soils.•Piezometers and tensiometers can be used to measure the in situ pore-water pressure at any point in a soil mass.•A piezometer can be used to measure positive pore-water pressures at a point in a soil mass.•A tensiometer can be used to measure negative pore-water pressures at a point in a soil mass.•In the above figure, point A has a higher total head than point B [i.e., hw (A) > h w (B)] and therefore water will flow from point A to point B due to the hydraulic head gradient.•The driving potential causing flow in the water phase through a soil mass has the same form for both saturated (i.e., point B ) and unsaturated (i.e., point A ) soils (Freeze & Cherry, 1979).Additional notes:•Darcy’s law describes the flow of water through an unsaturated soil. However, the coefficient of permeability in an unsaturated soil need not be a constant.•The coefficient of permeability in an unsaturated soil is a variable that is predominantly a function of the water content or the matric suction in the unsaturated soil.•Water can be visualized as flowing only through the pore space filled with water. The air filled pore spaces are nonconductive channels to the flow of water.•To verify Darcy’s law for unsaturated soils, experiments were conducted using acolumn of unsaturated soil, with a uniform water content and a constant water pressure head subjected to various gradients of gravitational heads.•The results indicated that at a specific water content, the coefficient of permeability, k w , is constant for various hydraulic head gradients applied to the unsaturated soil.•The rate of water flow through an unsaturated soil is linearly proportional to the hydraulic head gradient, with the coefficient of permeability being a constant, similar to the situation for a saturated soil.•Therefore, Darcy’s law can also be applied to unsaturated soils.However, themagnitude of the coefficient of permeability is a function of volumetric water content, θw .Additional notes:•The coefficient of permeability depends upon the properties of the fluid and the properties of the porous medium. Different types of fluids (e.g., water and oil) ordifferent types of soil (e.g., sand and clay) produce different values for the coefficient of permeability, k w .•The intrinsic permeability of a soil, K , represents the characteristics of the porous medium and is independent of the fluid properties.•In geotechnical engineering, the term coefficient of permeability, kw , is a commonly used term.•In an unsaturated soil, the coefficient of permeability, k w , is affected by changes in void ratio and more significantly by the degree of saturation (or water content).•As a soil becomes unsaturated, air first replaces some of the water in the large pores, and this causes water to flow through the smaller pores, with an increased tortuosity to the flow path.•Additional increases in matric suction leads to further decreases in the the pore volume occupied by water, and the air-water interface is drawn closer and closer to the soil particles.•As a result, the coefficient of permeability with respect to the water phase decreases rapidly as the space available for water flow reduces.Additional notes:•Change in void ratio in an unsaturated soil may be small. However, the effect of a change in degree of saturation may be highly significant.•As a result, the coefficient of permeability is often described as a singular function of the degree of saturation, S,or the volumetric water content, θw.•A change in matric suction can produce a more significant change in the degree of saturation than can be produced by a change in net normal stress.•Degree of saturation has been commonly described as a function of matric suction. •Numerous semi-empirical equations for the coefficient of permeability have been derived using the matric suction versus versus degree of saturation curve.•In these equations, the pore-size distribution forms the basis for predicting the coefficient of permeability.•The residual degree of saturation,Sr , is defined as the degree of saturation at whichan increase in matric suction does not produce a significant change in the degree of saturation.Additional notes:•The matric suction versus degree of saturation curve exhibits hysteresis and thedrainage curve is most commonly used. In addition, the soil structure is assumed to be incompressible.•The air entry value of the soil, (u a -u w )b , residual degree of saturation, S r , and the pore size distribution index, λ, are three parameters commonly used when deriving equations for predicting the coefficient of permeability, k w .•Using the concept of effective degree of saturation, S e , the above figure illustrates a procedure for determining the air entry value, the residual degree of saturation, and the pore size distribution index.•The effective degree of saturation can be computed by first estimating the residual degree of saturation.•Plotting the effective degree of saturation versus matric suction and adjusting a horizontal and a sloping line to the calculated data permit the use of a new assumed residual degree of saturation.•The procedure is repeated until all points on the sloping line constitute a straight line. The pore size distribution index, λ,is defined as the negative slope of the effective degree of saturation, S e , versus the matric suction, (u a -u w ), curve.•The intersection point between the straight sloping line and the saturation ordinate (i.e., S e = 1.0) defines the air entry value of the soil.Additional notes:•The above figure shows an equation for effective degree of saturation as a function of the matric suction for values of matric suction greater than the air entry value of the soil.•The figure also illustrates typical matric suction versus degree of saturation curves for various soils.Additional notes:•The above figure illustrates typical λvalues for various soils that have been obtained from matric suction versus degree of saturation curves.•Soils with a wide range of pore sizes have a small value for λ. The more uniform the distribution of pore sizes in a soil, the larger the value for λ.Additional notes:•Brooks and Corey (1964) proposed an equation for the coefficient of permeability, k w , by using the matric suction versus the degree of saturation curve and the concepts of effective degree of saturation and pore-size distribution index , λ.•The coefficient of permeability, kw , for a sandstone is expressed in terms of the relative permeability as compared to the coefficient of permeability of the soil at saturated conditions, k s .•The figure also presents the air permeability of the soil to illustrate its decreasing value as the soil approaches a degree of saturation equal to 100%.Additional notes:•The above table presents several empirical constant, δvalues (i.e., Brooks and Corey, 1964 parameter) and their corresponding pore size distribution indices,λ, for various soil types.(Note that δ= (2+3λ) / λ)•The above values can be used in practice as a first estimation for different soils.Additional notes:•The above figure presents the equation proposed by Gardner (1958) for predicting the coefficient of permeability for an unsaturated soil as function of matric suction.•The equation provides as flexible permeability function that is defined by two constants (a and n ) where “a”is related to the breaking point (i.e., air entry value) and “n ” is defined by the slope of the function.•Four typical functions, with differing values of “a”and “n”illustrates the forms produced by Gardner’s (1958) equation.Additional notes:Gardner’s equation(1958)•The above table summarizes several of the proposed relationships between the coefficient of permeability and matric suction.•The equations illustrate the relationship between coefficient of permeability and matric suction by using soil constants that can be evaluated from the matric suction versus degree of saturation curves.Additional notes:•A coefficient of permeability function, k w (θw ), was proposed using the configurations of the pore space filled with water (Childs and Collis-George, 1950).•The soil-water characteristic curve can also be presented in terms of volumetric water content, θw , versus the matric suction. Therefore, k w can also be expressed directly as a function of matric suction.•The permeability function, k w (θw ), is derived based on Poiseuille’s equation.•The above permeability function has a similar form to the function presented by Kunze et al., (1968) and is slightly modified to use SI units and matric suction is used in the equation instead of pore-water pressure head.•The calculation of the permeability function, k w (θw ),at a specific volumetric water content involves the summation of the matric suction values that correspond to the volumetric water content at and below the value under consideration, (θw )i .Additional notes:Childs andCollis-George(1950)•The calculation of the permeability function, k w (θw ),at a specific volumetric water content involves the summation of the matric suction values that correspond to the volumetric water content at and below the water content under consideration, (θw )i .•A matching factor, (k s /k sc ), based on the saturated coefficient of permeability is necessary in order to provide a more accurate representation of the unsaturated permeability function.•The shape of the permeability function is determined by the terms inside thesummation sign portion of the equation as obtained from the soil-water characteristic curve.•The magnitude of the permeability function needs to be adjusted with reference to the measured saturated coefficient of permeability, k s , by using the matching factor.•If the saturated coefficient of permeability is measured, the permeability function can be predicted directly from the soil-water characteristic curve because all of the terms in front of the summation sign in the equation can be considered as an adjusting factor.•Therefore, the permeability function, k w (θw ), can be written as shown at the bottom of the slide.Additional notes:Childs andCollis-George(1950)•The soil-water characteristic curve can be visualized as an indication of theconfiguration of water-filled pores in the soil mass.•The coefficient of permeability is obtained by dividing the soil-water characteristic curve into “m” equal intervals along the volumetric water content axis.•The matric suction corresponding to the midpoint of each interval is used to calculate the coefficient of permeability.•This computational procedure for obtaining the permeability function appears to be most successful for sandy soils having a narrow pore size distribution (Nielsen et al., 1972).•The soil-water characteristic curve and the prediction of the permeability function for a fine sand (Lakeland sand) are shown on the next slides.Additional notes:•The soil-water characteristic curve for the Lakeland fine sand is shown above. •The figure shows a low air entry value for the soil specimens tested [i.e., (u a -u w )b around 3 kPa] and an estimated residual degree of saturation around 10 kPa.Additional notes:•A comparison between the permeability function, kw (θw ),computed from theproposed equation and experimental data is shown for a fine sand.•There is a satisfactory match between the measured and calculated values.Additional notes:•The coefficient of permeability, k w , is generally assumed to be uniquely related to the degree of saturation, S , or the volumetric water content, θw .•This assumption is reasonable since water flows through the pore-water phase.•The degree of saturation or volumetric water content shows significant hysteresis when plotted versus matric suction.Additional notes:•The relationships between the water content (i.e., S or θw ) and thecoefficient of permeability appear to exhibit little hysteresis (Nielsen and Biggar, 1961).•The permeability function, k w (or θw ), for coarse-textured soils isapproximately the same for both wetting and drying (Nielsen et al., 1972.)Additional notes:No hysteresis•Since there is hysteresis in the relationship between water content and matricsuction, there is also hysteresis in the relationship between the coefficient ofpermeability and matric suction.•The hysteresis in the relationship between the coefficient of permeability andmatric suction depends upon the distribution of pores in the soil.Additional notes:•Air in an unsaturated soil can be present in two forms; namely, a continuous form or as occluded air bubbles.•Air phase is generally continuous when the degree of saturation is below 85%.•Air phase becomes occluded bubbles as the degree of saturation is higher than 90% and the air flow is reduced to a process of diffusion through the pore-water.•Concentration or pressure gradient can be used as the driving potential for the flow of the continuous air phase through an unsaturated soil.•Fick’s law is often used to describe the diffusion of gases through liquids.•A modified Fick’s law, as shown above, relates the mass rate of flow, Ja , to theconcentration gradient, (dC /dy ), by using the transmission constant for air flow through a soil, D a .•The negative sign in the relationship indicates that air flows in the direction of a decreasing concentration gradient.•The concentration of the air, C , in a soil can be expressed as a function of the air pressure by using the air density which is related to the absolute air pressure in accordance with the gas law.Additional notes:•The modified Fick’s law, as shown above, can be used to express the mass rate of flow, Ja , to the pore-air pressure gradient, (d ua /d y ), by using a modified coefficient oftransmission for air flow through soils,D a *.•It can be observed that D a *is a function of the volume-mass properties of the soil.•This modified form of Fick’s law has been used in geotechnical engineering to describe air flow through soils (Blight, 1971).•Air flow, under steady-state conditions, can be measured through a soil mass. At the exit point, the mass rate of the air flow is measured at a constant air density, ρma .•This particular steady-steady condition permits the establishment of a relationship between the flow rate, v a , and the air pressure gradient, d u a /d y .Additional notes:•The pore-air pressure, u a , can be expressed in terms of pore-air pressure head, h a , using a constant air density (i.e., by neglecting the elevation head gradient).•Therefore, the equation for air flow through an unsaturated soil mass has the same form as Darcy’s equation for air phase, when the term k a is used for D a * g , and the hydraulic head gradient consists of the pore-air pressure head gradient as the driving potential.•The coefficient of permeability for the air phase is a function of the fluid (i.e., air) and the soil volume-mass properties.•Air flows through the air phase and as the matric suction increases, or the degree of saturation increases and the air coefficient of permeability increases.•Relationships between air coefficient of permeability and matric suction or degree ofsaturation reflect the pore size distribution and the matric suction versus degree of saturation curve of the soil.•The relationship at the bottom is obtained when the effective degree of saturation, S e , is expressed in terms of matric suction.•The air coefficient of permeability, k a , is essentially the inverse of the water coefficient of permeability function, k w .(Brooks and Corey, 1964)Additional notes:•The relationships between ka and degree of saturation or matric suction are also validfor the air coefficient of transmissivity, Da *, since the two coefficients are related bythe constant, “g”.•Experimental verifications have been performed for Fick’s and Da1971) and some results are presented.•The tests were performed by establishing steady-state air flows through dry soils.The soils were assumed to have a rigid structure because no measurable volume change occurred during the tests.•In order to use the bulk soil as a reference, the mass rate of air flow must be multiplied by the air porosity, na.•The above figure illustrates the applicability of Fick’s law to air flow. For a small change in the pore-air pressure gradient, the mass flow rate,J, is almost linearlyproportional to the pore-air pressure gradient, with Da * being the coefficient ofproportionality.•The air pressure gradients used in the experiments were high, and the pressures applied could affect the soil structure (i.e., altering the pore-soil distribution) and themagnitudes for both the Da * and kacoefficients.Additional notes:Fick’s Law•The above figure illustrates the applicability of Darcy’s law to air flow. For a smallchange in the pore-air head gradient, the flow rate of air, va , is almost linearlyproportional to the pore-air head gradient, with ka being the coefficient ofproportionality.•The above results were calculated using a constant air density, ρa , at a pressure of 101.3kPa and a temperature of 20 o C (i.e., 1.20 kg/m3). Additional notes:Darcy’s Law•The above figure illustrates the agreement between measured data and the theoretical air coefficient of permeability function by using the air permeability equation defined with effective degree of saturation expressed in terms of matric suction.•The results are presented in terms of relative permeability with the maximum air coefficient of permeability corresponding to the dry soil conditions.Additional notes:•The above figure presents air and water coefficients of permeability for a soilcompacted at different water contents or matric suction values.•The air coefficient of permeability, ka , decreases as the soil water content ordegree of saturation decreases (Ladd, 1960; Barden and Pavlakis,1971).•The air and the water coefficients of permeability were measured on the same soil specimens under steady-state flow conditions induced by small pressuregradients applied to each phase.•The air coefficient of permeability , ka , decreases rapidly as optimum watercontent is approached. At this point, the air phase becomes occluded and the flow of air takes place as diffusion of air through water.•The coefficient of permeability decreases and the water coefficient ofpermeability increases with increasing water content, however, the aircoefficients of permeability remain significantly greater than the watercoefficients of permeability.•The difference in air and water viscosities (i.e., water viscosity is about 56 times the air viscosity at atmospheric conditions) is one of the reasons for the aircoefficient of permeability being greater than the water coefficient ofpermeability.Additional notes:•The diffusion process occurs in response to a concentration gradient. Ionic or molecular movement take place from regions of higher concentration to regions of lower concentration.•There are two common diffusion mechanisms of interest in unsaturated soil mechanics, the first is the flow of air through the pore-water in a saturated or unsaturated soil, the second process is related to air flow through the water in a high air entry ceramic disk. •Fick’s law, which is shown above, is often used to describe the first diffusion process. The concentration gradient provides the driving potential for the diffusion process and is expressed with respect to the soil voids (i.e., air and water phases).•Fick’s law equation can also be written in terms of the partial pressure of a constituent gas diffusing through the pore-water in a soil, by expressing the concentration of the gas constituents in terms of partial pressures.Additional notes:•The mass rate of the gas constituents diffusing across a unit area of the soil voids (i.e., J a ) can be determined by measuring the volume of the diffused constituents under constant pressure conditions.•The ideal gas law is applied to the diffusing constituents.•A change in concentration of the diffusing constituent relative to a change in partial pressure is obtained by using the change in density of the dissolved constituent in the pore-water.•Using the ideal gas law yields the equation at the bottom of the slide which expresses the change in concentration of the diffusing gas in terms of the partial pressure of the diffusing constituent and the volume of the dissolved constituent, V d i , through the pore-water.Additional notes:•The ratio of the volume of dissolved constituent to the volume of water, (i.e., V di /V w ) is referred to as the volumetric coefficient of solubility, h .•The flow rate of the diffusing constituent across a unit area of the soil voids, v f i , can beexpressed as a function of the partial pressure gradient, (i.e.,in this case in the y-direction) by combining the previous equations.•The equation for the diffusing constituent, vf i , can be applied to air or gas diffusion through the pore-water in a soil or through free water or some other material such as a rubber membrane (Poulos, 1964).•The equation for the diffusing constituent, v fi , can also be developed in terms of the partial pressure head, h fi , with respect to the constituent density ρfi , which corresponds to the absolute constant pressure,u fi , used in the measurement of the diffusing constituent volume.•By defining the diffusion coefficient of permeability for air through an unsaturated soil with occluded air bubbles, the equation for the flow rate of the diffusing gas can be expressed as a modified form of Darcy’s law, as shown at the bottom of the slide.Additional notes:。

非饱和土力学ppt课件.ppt

已提出了由干燥曲线预测增湿曲线，或由增湿曲线预测干燥曲线的方法（Phan H。Q，2003)
• 非饱和土基本特性的学习／2、非饱和土的吸力特性
土－水特征曲线形态的重要参数
由于土中的水分可以有结晶水、吸着水、结合水（薄膜水）和自由水等
具有不同属性的不同类型。含水量变化时，土中水有不同的类型，气有不同的连通，
孔隙水压力和孔隙气压力分别在土的孔隙水体和孔隙气体中是各向等压的静水压力型应力
孔隙水压力和孔隙气压力各自作用在其与土颗粒接触部分的表面上，其差值对土骨架的作用不会是各处相等的。
当孔隙水为弯液面环状水时，吸力只在接触点的法向上作用；当孔隙水为有弯液面的体积水时，所产生的吸力必然有法向和切向两个方向上分力的作用。国内也出现了湿吸力与牵引力的提法（汤连生）。
单一有效应力型的应力状态变量
人们在寻求非饱和土的应力状态变量时，首先想到了单一有效应力型的应力状态变量
它不是一般的纯力学量，而是一个材料有关的力学量，与材料的本构关系有着密切的联系（如饱和土力学中的有效应力）。研究提出具有真实合理性的有效应力表达式是当前的主要任务。
对已经提出的各种表达式还需要作出认真的选择与检验。
导致了非饱和土十分复杂的力学性质。
• 非饱和土基本特性的学习／2、非饱和土的吸力特性
2、非饱和土的吸力特性
非饱和土的土水势一般包括温度势、压力势、重力势、基质势和溶质势
在等温、等压、等高（不计重力）的情况下，土中水的温度势、压力势、重力势保持不变，
自由能的变化只有基质势和溶质势的变化。
如将它们分别称之为基质吸力和溶质吸力，它们之和，即此时的自由能，称为总吸力，则有
应该取决于各自的相对压缩性。
在孔隙流体不能排出的条件下，土受力后的孔隙水压力和孔隙气压力的增量是一种超孔隙压力

非饱和土状态相关本构关系及应用-哈尔滨工业大学深圳

(3)发现了吸力影响土体结构和大应变剪胀（缩）的规律，推导了吸力状态相关的大应变剪胀（缩）本构关系，利用自主研发的双室高精度体变测量系统在国际上率先验证了吸力增加土体剪胀势，突破了经典非饱和土应力-应变本构关系忽略吸力状态效应的局限性。
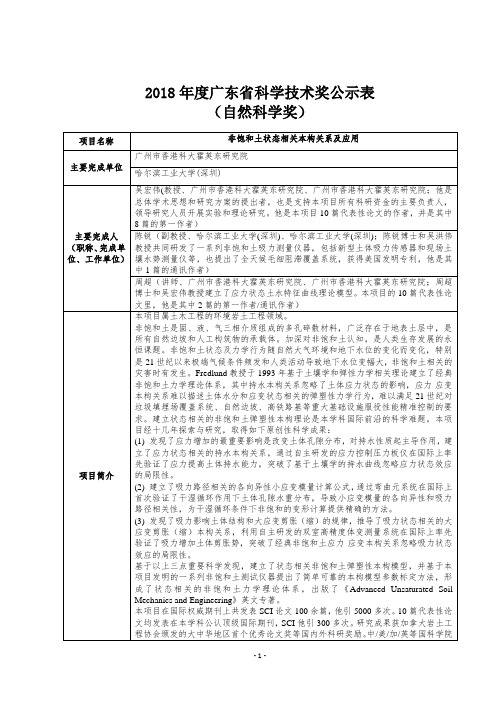
基于以上三点重要科学发现，建立了状态相关非饱和土弹塑性本构模型，并基于本项目发明的一系列非饱和土测试仪器提出了简单可靠的本构模型参数标定方法，形成了状态相关的非饱和土力学理论体系，出版了《Advanced Unsaturated Soil Mechanics and Engineering》英文专著。
知识产权名称
专利1：All-weather landfill soil cover system for preventing water infiltration and landfill gas emission（US9101968 B2）
专利2：用于土工离心机中模拟植物蒸腾作用的试验装置及方法（CN103424535B）
论文5：A state-dependent elasto-plastic model for saturated and unsaturated soils
论文6：Performance of an unsaturated expansive soil slope subjected to artificial rainfall infiltration
陈锐（副教授、哈尔滨工业大学(深圳)、哈尔滨工业大学(深圳)；陈锐博士和吴洪伟教授共同研发了一系列非饱和土吸力测量仪器，包括新型土体吸力传感器和现场土壤水势测量仪等，也提出了全天候毛细阻滞覆盖系统，获得美国发明专利，他是其中1篇的通讯作者）
周超（讲师、广州市香港科大霍英东研究院、广州市香港科大霍英东研究院；周超博士和吴宏伟教授建立了应力状态土水特征曲线理论模型。本项目的10篇代表性论文里，他是其中2篇的第一作者/通讯作者）

非饱和土土力学新PPT课件

强度理论与本构模型
库仑公式本构模型
D uf can－cChantgg模型，剑桥模型
屈服面
极限状态面
第3页/共54页
传统（经典）土力学的局限
固结理论
pw
1-D consolidation
k 2 pw pw
wm z2 t
(Lambe & Whitman,1969)
3-D consolidation
2 4 6 8 10 12 14 16 18
第24页/共54页
吸力的影响
温度的影响
/kPa
非饱和土土力学理论
4、非饱和土的应力应变关系及本构模型
（1）弹性模型 Fredlund和Morganstern(1976)、Fredlund(1979)提出了基于双应力变量 ( ua ) 和 (ua uw )
第11页/共54页
非饱和土土力学理论
吸力随含水量变化，其表现形式不同
在第Ⅰ阶段，土体处于饱和状态，土孔隙中为能够传递压力的自由水，没有水气接触面存在，也没有由表面张力产生的毛细应力。
第Ⅱ阶段，为毛细作用发挥阶段。当基质吸力超过最大空气进气值，土体开始进入非饱和状态，含水率从饱和含水率变化到塑限含水率，毛细应力开始快速增加。
w ( p)
第26页/共54页
非饱和土土力学理论
4、非饱和土的应力应变关系及本构模型
（3）弹塑性模型（陈正汉）
屈服点的轨迹在p-s面内是一条曲线，在 p-q面内随吸力增加向外扩展，据此构建非饱和土的统一屈服面模型。
p0 p0* ms n[e / patm 1]
吸力的影响
吸力 s(kPa)
200
k wm
2
pw

非饱和红土邓肯-张模型参数的试验研究

非饱和红土邓肯-张模型参数的试验研究刘星志;刘小文;张占磊;周龙【摘要】According to the derivation method of nonlinear constitutive model for unsaturated soil,the Duncan-Chang parameters(Es,Bst,Kw)of unsaturated laterite under the compaction degree of 0. 9 were deduced by GDS triaxial consolidated drained tests. At the same time,combined with the GDS triaxial instrument for wetting deforma-tion test of gradually increasing humidity under different stress levels,the soil tangent bulk modulus associated with the matrix suction( Hs )and the bulk modulus of water( Hw )in Duncan-Chang were obtained. Then,the related parameters of nonlinear constitutive model for unsaturated laterite were proposed,which provided a basis for the non-linear constitutive relation of Duncan-Chang model for unsaturated laterite.%根据Fredlund D等提出的非饱和土的非线性本构模型推导方法,通过GDS三轴固结排水试验推导出了压实度为0.9下非饱和红土的邓肯-张Es、Bst、Kw参数,同时结合GDS三轴仪进行不同应力水平下的逐渐增湿湿化变形试验,得到邓肯-张模型中与基质吸力相关的土切线体积模量Hs和水的体积模量Hw.由此提出了非饱和红土的非线性本构模型的相关参数,为扩展的非饱和红土邓肯-张模型的非线性本构关系的有限元编程提供了依据.【期刊名称】《南昌大学学报（工科版）》【年(卷),期】2016(038)004【总页数】7页(P347-353)【关键词】非饱和红土;邓肯-张模型;GDS三轴试验;参数【作者】刘星志;刘小文;张占磊;周龙【作者单位】南昌大学建筑工程学院,江西南昌330031;南昌大学建筑工程学院,江西南昌330031;南昌大学建筑工程学院,江西南昌330031;南昌大学建筑工程学院,江西南昌330031【正文语种】中文【中图分类】TU411红土在江西地区分布十分广泛，道路路基、房屋地基、河流堤坡等很多实际工程都位于红土地区，且这些红土地区的土大多处于非饱和状态，因此对江西非饱和红土的力学特性及本构关系研究非常有必要[1]。

近代科学之父牛顿 ppt课件

的时候没有得到什么特殊的荣誉。
2021/3/30
11
斯蒂克利（第一个写牛顿传记的作者）记述：
“牛顿爵士在接受文学学士学位的仪式上被安排在第二列，属于人们戏称的输掉银币者”。（“输掉银币者”是指当时的一个传统：学生要向考试官缴纳9枚银币，如果学生考得好，考完之后银币发还给他；如果考得不好，钱就没收了。）
经典力学奠基者（17世纪初）
伽利略（意）
2021/3/30
《自然哲学中的数学原理》
经典力学建立者（17世纪晚期）
从经典力学发展到相对论、量子论（19末20初）
牛顿（英）
爱因斯坦（德、美）普朗克（德）
22
历史上证实牛顿力学的三件事
2021/3/30
第一件，用牛顿力学算出地球的形状象只桔子。
在剑桥大学，牛顿开始既不出色，也没有引起教授
的注意。牛顿是一个向来专心用功的学生，大学的必修
课程也要求十分紧迫，可是他的努力却大都花费在必修
课程以外的知识上。牛顿和达尔文、爱因斯坦、霍金以
及另外一些牛顿之后的伟大科学家一样，对于作为一个
好学生所必须通过的许多考试，往往会准备不足。牛顿
在考试所需复习的正式课程方面不甚用功，结果他毕业
第二件，用牛顿力学算出了哈雷彗星回归周期
长期以来到，人们一直认为彗星是神秘的，根本不能
用科学来说明。牛顿说，既然彗星也是天体，就必然遵循
力学规律。他的好友哈雷用牛顿力学算出了1682年出现
的彗星的轨道，指出它的回归周期是75—76年，由此预
言它将在1758年再次出现。哈雷1705年发表这个预言，
当时他已49岁，却要预言53年以后的事，岂非耸人听闻？
26
牛顿的主要科学成就

关于牛顿的讲座ppt讲解

就：微积分、万有引力、光学分析的思想就是在这时孕育成形的。可以说此时的牛顿已经开始着手描绘他一生大多数科学创造的蓝图。
鹿胎膏 /
员工自评范文 /
二、科学成就
1、数学(大学毕业前)：
提出二项式定理以及微积分的初步算法
2、天文学：
英国诗人波普曾经这样写道：
自然界和自然规律隐藏在黑暗中，上帝说：让牛顿出生吧！于是，一切都成为光明。
一、成长的足迹
牛顿出生前，父亲已去世。牛顿是遗腹子，又是早产儿，先天不足，出生时体重只有3磅（1.36公斤），差点夭折。他两岁时母亲改嫁，靠外祖母抚养。牛顿小学时期，体弱多病，性格腼腆，生性孱弱，有些迟钝，自幼沉默寡言，性格倔强，而这种习性可能来自他的家庭处境。
牛顿自小是个孤儿，爱好单调，性格孤僻，脾气暴躁，工作有偏致狂倾向。他在科学上做出了无与伦比的贡献，但在处理人事关系上做的极不好，一生没有一个挚友，一生没有结婚。牛顿一直在孤独中度过自己人生，可能只有在教堂里同上帝对话才能使自己心理得到一些宽慰。
牛顿的科学成就
1.力学方面：（1）牛顿总结出了物体运动的三个基本定律。（2）牛顿发现了万有引力定律。
究专心到痴情的地步。据说有一次牛顿煮鸡蛋，他一边看书一边干活，糊里糊涂地把一块怀表扔进了锅里，等水煮开后，揭盖一看，才知道错把怀表当鸡蛋煮了。
29
牛顿轶事：
3、有一次，他在向一位姑娘求婚时思想又开了小差，他脑海了只剩下了无穷量的二项式定理。他抓住姑娘的手指，错误的把它当成通烟斗的通条，硬往烟斗里塞，痛得姑娘大叫，离他而去。牛顿也因此终生未娶。
然而他却并不是天生的神童。今天我们就来了解这位伟大的科学家的一生，寻觅他行程的足迹，揭示这位智慧巨匠崇高的人格魅力。

降雨入渗条件下非饱和土边坡稳定性研究进展

降雨入渗条件下非饱和土边坡稳定性研究进展刘翠;徐文成【摘要】Rainfall, especially the long-lasting heavy rainstorm, is a major cause of instability and failure of slope. During the rainfall, a large number of rain infiltrations will cause the slope soil saturation in-creases, the matrices suction of non-saturated region reduces and the soil shear strength decreases. When the rainfall intensity and duration exceeds a certain extent, it may lead to slope instability. Based on the discussion of unsaturated soil strength theory and percolation theory, the progress of unsaturated soil slope stability research in recent years was discussed.%降雨，特别是强度大、历时长的暴雨，是引起边坡失稳破坏的主要因素。

降雨期间，雨水的大量入渗使边坡土体的饱和度增加，非饱和区基质吸力降低，土体抗剪强度下降。

当降雨的强度和持续时间超过一定程度时，便可能导致边坡失稳。

在讨论非饱和土强度理论和渗流理论的基础上，探讨了近年来关于非饱和土边坡稳定性研究的进展。

【期刊名称】《黄河水利职业技术学院学报》【年(卷),期】2015(000)003【总页数】4页(P24-27)【关键词】边坡稳定性;非饱和土;降雨入渗【作者】刘翠;徐文成【作者单位】黄河水利职业技术学院，河南开封 475004;河南省豫东水利工程管理局，河南开封 475002【正文语种】中文【中图分类】TU457边坡的失稳破坏和降雨是人类生活中常见的事物，自然规律的作用成了两者紧密联系的桥梁。