Global properties of static spherically symmetric charged dilaton spacetimes with a Liouvil
国际标准层型剖面及点位表GSSPTable
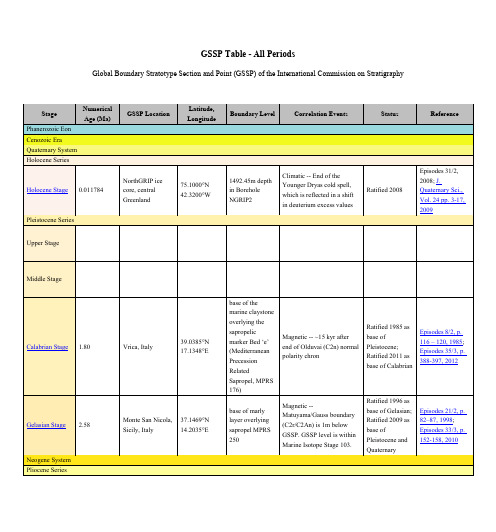
Oxygen isotopic event Mi-1.
Possibly Monte Cagnero, Umbria-Marche region, Italy
Zumaia section, northern Spain
Gorrondatxe section, Basque Country, Spain
Tiziano Bed
43°22'46.47"N, 3°00'51.61"W
dark marl at 167.85 m in Gorrondatxe sea-cliff section
Calcareous nannofossil near FAD Chiasmolithus oamaruensis (base Zone NP18)
GSSP Table - All Periods
Global Boundary Stratotype Section and Point (GSSP) of the International Commission on Stratigraphy
Stage
Numerical Age (Ma)
Phanerozoic Eon
Vrica, Italy
39.0385°N 17.1348°E
Monte San Nicola, 37.1469°N
Sicily, Italy
14.2035°E
base of the marine claystone overlying the sapropelic marker Bed ‘e’ (Mediterranean Precession Related Sapropel, MPRS 176)
Optical scattering properties of organic-rich and inorganic-rich particles in inland waters

Optical scattering properties of organic-rich and inorganic-rich particles in inland watersKun Shi a ,b ,c ,Yunmei Li b ,⁎,Yunlin Zhang a ,Lin Li c ,Heng Lv b ,Kaishan Song daTaihu Lake Laboratory Ecosystem Station,State Key Laboratory of Lake Science and Environment,Nanjing Institute of Geography and Limnology,Chinese Academy of Sciences,Jiangsu Nanjing 210008China bKey Laboratory of Virtual Geographic Environment,Ministry of Education,College of Geographic Sciences,Nanjing Normal University,Nanjing 210046China cDepartment of Earth Science,Indiana University –Purdue University Indianapolis,723West Michigan Street,SL118,Indianapolis,IN 46202USA dNortheast Institute of Geography and Agricultural Ecology,Chinese Academy of Sciences,Changchun Jilin 130012Chinaa b s t r a c ta r t i c l e i n f o Article history:Received 19May 2013Accepted 1February 2014Available online 25March 2014Communicated by Barry Lesht Index words:Lake TaihuScattering coef ficientMass-speci fic scattering coef ficient PhytoplanktonWe present the results from a study of the particulate scattering properties of three bodies of water that represent a wide range of optical properties found in inland waters.We found a positive linear relationship (R 2=0.45,P b 0.005)between the mass-speci fic scattering coef ficient at 532nm (b p *(532))and the ratio of the inorganic suspended material (ISM)to the total suspended material (TSM)in our study areas.In con-trast to earlier studies in which b p *(532)was lower for inorganic particles than for organic particles,we found that the value of b p *(532)for ISM (b p *(532)ISM =0.71m 2/g)was approximately 1.6times greater than the value found for organic suspended materials (OSM)(b p *(532)OSM =0.45m 2/g).We found that the dependence of the particle scattering coef ficient (b p )on wavelength (λ)could be described accurately by a power law (with mean average percent error (MAPE)b 0.07)in waters dominated by inorganic particles.The model errors in waters dominated by organic particles,however,were much larger (MAPE N 0.1),especially in the spectral region associated with strong phytoplankton absorption.The errors could be reduced over this wavelength range by adding a term to the model to account for particle absorption,but the additional term tended to increase the error outside of this range.We conclude that information about the nature of the scatter-ing particles in lake waters is necessary for the selection of an appropriate model for particle absorption and that a hybrid model that includes absorption over some wavelength ranges may be necessary.©2014International Association for Great Lakes Research.Published by Elsevier B.V.All rights reserved.IntroductionInherent optical properties (IOPs)of water are solely dependent on the water contents,such as the concentrations of dissolved water con-stituents and particulate composition,but independent of the distribu-tion of ambient light (Morel,1988).Light scattering is generally considered one of the most fundamental parameters of IOPs and can re-flect the composition and shape characteristics of the total suspended materials (Loisel et al.,2006).Therefore,particulate scattering and a de-tailed understanding of its variability in natural waters are important for aquatic ecosystem sciences related to the knowledge of total suspended materials.The scattering properties of a water body can determine the way light propagates through water,and this information can be used for inferring water contents from data observed using remote sensing systems (Snyder et al.,2008).In turbid inland waters,scattering is very important in the remote sensing of water contents because theradiometric signal recorded by a sensor onboard a satellite or an aircraft is directly proportional to its intensity (Twardowski et al.,2001).The majority of scattering is composed of total suspended material (TSM),including organic suspended material (OSM)and inorganic suspended material (ISM).Particulate scattering has been found to be directly associated with the TSM concentration,but the relationship be-tween them varies with the composition of rge differences in TSM compositions are found in different water types which results in signi ficant variation in the speci fic scattering coef ficient.As theoretical-ly expected,the relationship between the scattering,b p (λ),and the con-centration of suspended particles was observed to change signi ficantly with the particle size distribution and refractive index (Babin et al.,2003).Babin et al.(2003)argued that the mass-speci fic coef ficient in Case 2waters with a high inorganic content would be smaller than in Case 1waters with a high organic content.Baker and Lavelle (1984)also suggested that a systematic decrease in the mass-speci fic scattering coef ficient could occur from offshore to inshore waters.The in fluence of the mass-speci fic scattering coef ficient would also be reduced by algal absorption in areas with a high chlorophyll-a concentration (Chl-a ).On the basis of theoretical considerations,Morel (1988)showed that spectral variations of the scattering coef ficient caused by non-absorbingJournal of Great Lakes Research 40(2014)308–316⁎Corresponding author.Tel.:+862585898500.E-mail address:yunmeinjnu@ (Y.Li)./10.1016/j.jglr.2014.02.0220380-1330/©2014International Association for Great Lakes Research.Published by Elsevier B.V.All rightsreserved.Contents lists available at ScienceDirectJournal of Great Lakes Researchj o u r n a l h o me p a g e :ww w.e l s e v i e r.c o m /l o c a t e /j gl rparticles follow an inverse power law.This dependency has been used in models of the inherent properties of seawater and inland lake waters (Babin et al.,2003;Morel et al.,2006;Roesler and Boss,2003;Song and Tang,2006;Sun et al.,2009).Based on the observations made in a num-ber of estuaries,Doxaran et al.(2009)confirmed that,in the near-IR spectral region,where light absorption by particles is low,a power law function doesfit the spectral variations in a scattering coefficient with a variable slope.However,this power law function is inappropriate for the visible region of the spectrum where particles absorb more light (Doxaran et al.,2007).Numerous measurements of spectral scattering coefficients have documented significant departures from the power law function in spectral bands associated with strong particulate ab-sorption(Babin et al.,2003;Barnard et al.,1998;Doxaran et al.,2007; Stramski et al.,2001).In Lake Taihu,due to the low OSM to ISM ratio, a power law function is suitable for the spectral variations in a scattering coefficient with variable slope.Therefore,the impact of OSM absorption on the scattering coefficient is negligible in Lake Taihu(Sun et al.,2009). When the TSM has a high OSM ratio,the model canfit spectral varia-tions in the scattering coefficients with variable slopes in wave bands associated with strong particulate absorption by taking into account the particulate absorption effects(Doxaran et al.,2009).For waters with high inorganic particle contents,numerous studies have been conducted to develop models that simulate the variations in spectral scattering coefficients and to investigate the variations in the relationship between scattering coefficients and TSM and ISM (Boss et al.,2004;Kirk,1981;Loisel and Stramski,2000;Loisel et al., 2006;Whitmire et al.,2007).However,little work has been performed on productive inland waters with high organic particle contents.This study focuses on the properties of optical scattering in three op-tically distinct regions in China:Lake Taihu,Lake Chaohu,and Lake Dianchi.The two questions we address in this study are(1)how well does a power-law function describe the shape of the particulate scatter-ing spectra in different inland waters(that is,waters with inorganic-rich or organic-rich particles)and(2)can the mass-specific scattering coefficient be related to the amount of organic and inorganic suspended materials?Material and methodsStudy areasThe study areas,including Lake Taihu,Lake Chaohu,and Lake Dianchi,are located in the Yangtze River drainage area(Fig.1).Lake Taihu is a large,eutrophic shallow lake with high spatial heterogeneity in the Yangtze Delta plain on the border of the Jiangsu and Zhejiang provinces in China.With an area of2338km2(Zhang et al.,2007),it is the third largest freshwater lake in China.This lake is a typical large shallow lake with an average depth of1.9m,indicating wave-induced sediment resuspension has a significant impact on the water quality of Lake ke Chaohu is located at the juncture of Chaohu and Hefei cities in Anhui Province,China.With a water area of750km2 and an average depth of3m,it is the largest lake in Anhui and one of thefive largest freshwater lakes in China.Similar to Lake Taihu,the water quality of this lake is also severely affected by wave-induced sed-iment resuspension.The optical properties of these two lakes(Lake Taihu and Lake Chaohu)are generally dominated by inorganic particles from resuspended bottom materials and a strong influence from gelbstoff(chromophoric dissolved organic matter;CDOM)with a rela-tively lower contribution from phytoplankton.Our third study site was Lake Dianchi,located on the Yungui Plateau in China.With a water area of approximately300km2and an average depth of5m,Lake Dianchi is the largest freshwater lake in the Yunnan Province.The optical properties of this lake were typically controlled by phytoplankton with minor influences from inorganic suspended mate-rials(Sun et al.,2012).These lakes have high concentrations of TSM.However,the TSM composition varied drastically among the three lakes.The waters in Lake Taihu and Lake Chaohu had a high concentration of non-algal par-ticles,i.e.,fine sediments with a combination of silts and clays.Con-versely,there was a high concentration of algal particles in Lake Dianchi.The measurements at Lake Taihu were performed in November 2008(56stations)and April2009(31stations).The measurements at Lakes Chaohu and Dianchi were carried out in June(30stations)and Fig.1.Geo-location of the three study lakes in China.309 K.Shi et al./Journal of Great Lakes Research40(2014)308–316September (25stations)2009,respectively.At each station,optical measurements were conducted on the surface water,and water sam-ples were collected using Niskin bottles.The samples were immediately preserved at a low temperature and taken to a laboratory for analysis that day.Optical parameter measurementsThe particulate absorption coef ficients and attenuation coef ficients were measured at a spectral resolution of 4nm and a measurement ac-curacy of ±0.01m −1using a WETLabs AC-S (WETLabs,Inc.,Philomath,OR)designed with 85spectral channels.To obtain accurate absorption and attenuation data,temperature corrections were performed using Eq.(1)(Sun et al.,2009,2010).The salinity correction is not necessary in these freshwater lakes.In addition,the third method of Zaneveld et al.(1994)was used to correct for particulate absorption and scatter-ing errors using spectral scattering and the measured value at the termi-nal wavelength of the AC-S (Eq.(2)).a mts λðÞ¼a m λðÞ−ψt Ãt −t r ðÞð1Þa pg λðÞ¼a mts λðÞ−a mts λrefð2Þwhere a m is the absorption coef ficient measured from the WETLabs AC-S,ψt is the temperature correction parameter,t is temperature of the water,a mts is the absorption coef ficient after the temperature correction,λdenotes the wavelength at which the corrected value is calculated,λref is the reference wavelength (715nm)at which a pg is assumed to be zero,and a pg is the absorption coef ficient of the particles and gelbstoff after the scattering correction.Therefore,the attenuation coef ficient of the particulates and gelbstoff (c pg (λ))could be calculated from:cpg (λ)=c m (λ)−ψt *(t −t r )‐amts (λref ),where c m (λ)is the attenuation coef ficient measured using the WETLabs AC-S.Because the scattering coef ficient of gelbstoff was low and therefore could be ignored,the particulate scattering coef ficients (b p (λ))could be obtained from the difference between the attenuation and absorption (Eq.(3)).bp λðÞ¼cpg λðÞ−apg λðÞð3Þwhere b p (λ)is the particulate scattering coef ficient,c pg (λ)is the atten-uation coef ficient of the particulates and gelbstoff,and λis wavelength.Water-component concentration measurementsThe water-component concentrations were measured according to the investigation criteria for lakes in China (Sun et al.,2009).The TSM,OSM,and ISM were measured using a weighing method.Chl-a was ex-tracted with hot ethanol (90%)at 82°C and analyzed spectrophotomet-rically with a correction for phaeopigments.The detailed procedures can be found in Le et al.(2009a).ResultsCharacteristics of water constituent concentrationsThe water component (TSM,ISM,OSM,and Chl-a )concentra-tions and the ratio,ISM/TSM,covered the wide range (TSM:11.4–237.7mg/L;ISM:0–214.87mg/L;Chl-a :3–192.9mg/m 3,and ISM/TSM:0–90%)in the various study areas (Table 1).The highest aver-age values of TSM and ISM concentrations were both found in Lake Taihu.The lowest average values of TSM and ISM concentrations were found in Lake Chaohu and Lake Dianchi,respectively.Thehighest and lowest average values of Chl-a were found in Lake Dianchi (97.3mg/m 3)and Lake Taihu (13.5mg/m 3),respectively.The water in Lake Taihu was highly turbid judging from the variation in TSM concentration,which was 11.4–237.7mg/L,with an average of 56.5mg/pared with other studies,such as sandpit lakes (Dall'Olmo and Gitelson,2005),Chesapeake Bay (Gitelson et al.,2008)and a number of other US lakes (Gitelson et al.,2009),the average con-centrations in our study areas were much higher.A high organic content was observed in Lake Dianchi (from 40%to 100%,with an average value of 80%of the TSM from organic matter).The suspended materials in Lake Chaohu showed a rather high organic content (on average,30%of the suspended materials were organic).In Lake Taihu (both autumn and spring),the TSM was mainly inorganic (from 40%to 90%,with an average of 80%,of the total mass of suspended materials)which has been reported in many studies (Binding et al.,2005;Boss et al.,2001a,b,2004;Bowers and Mitchelson-Jacob,1996;Bricaud and Morel,1986;Bricaud et al.,1998,2007;Clavano et al.,2007;Liu et al.,2004;Ma,2005;Morel,1973;Morel et al.,2007).The resulting dataset can be representative of various turbid,productive,inland waters and various types of particles.Characteristics of optical scattering coef ficientsThe statistics of the b p (λ)values are shown in Table 2.The highest (47.9m −1at 715nm)and lowest (1.8m −1at 715nm)b p (λ)values were found in Lake Taihu in autumn and spring,rgeTable 1Maximum (Max),minimum (Min),average,and standard deviation (SD)of the measured parameters chlorophyll a (Chl-a ),total suspended matter (TSM),inorganic suspended matter (ISM)and the ratio ISM/TSM)in the three lakes studied.Study areas parameters Max Min Average ke ChaohuChl-a (mg/m 3)192.923.862.443.2TSM (mg/L)83.915.743.617.1ISM (mg/L)68.48.430.114.3ISM/TSM0.80.40.70.1Lake TaihuChl-a (mg/m 3)79.8 3.013.514.6TSM (mg/L)237.711.456.548.8ISM (mg/L)214.8 6.046.744.9ISM/TSM0.90.50.80.1Lake DianchiChl-a (mg/m 3)156.739.097.334.6TSM (mg/L)66.624.745.09.7ISM (mg/L)22.80.08.9 4.1ISM/TSM0.60.00.20.1Table 2Statistics of the b p (λ)values obtained from the AC-S measurements at selected visible and near-IR wavelengths.Normality of the distributions was veri fied successfully using a Kolmogorov –Smirnov test on log-transformed data.The geometric standard devia-tion (SD)is to be applied as a factor.Study areasb p (m −1)Wavelength (nm)Max Min Average ke Taihu (autumn)53244.2 2.219.810.667539.5 1.815.88.671538.4 1.8158.1Lake Taihu (spring)53255.9102911.267549.68.524.29.671547.98.123.49.2Lake Dianchi53226.111.517 3.367521.89.614.1 2.871522.29.514.3 2.9Lake Chaohu53255.61228.712.167546.29.322.910.271544.39.122.19.8310K.Shi et al./Journal of Great Lakes Research 40(2014)308–316variations were observed in Lake Taihu and Lake Chaohu.The b p (λ)values decreased with increasing wavelength (Fig.2)as observed in the previous studies (Chami et al.,2005,2006;Claustre et al.,1999;Dall'Olmo and Gitelson,2005;Doxaran et al.,2009;Le et al.,2009b;Morel et al.,2006;Sun et al.,2009,2010).Table 3shows the correlation coef ficients between b p (532)and TSM,ISM and OSM.In Lake Taihu and Lake Chaohu,the coef ficients between b p (532)and both TSM and ISM are higher than between b p (532)and OSM.However,for Lake Dianchi,the relationship between b p (532)and OSM is closer than that between b p (532)and ISM.The correlation coef ficients between b p (532)and ISM are 0.95and 0.97in Lake Chaohu and Taihu while the coef ficient at Lake Dianchi is only 0.55,which indi-cates that inorganic particles dominate the water scattering characteris-tics at Lake Taihu and Lake Chaohu.At Lake Dianchi,the scattering characteristics are dominated by organic particles.The mass-speci fic particulate scattering coef ficient (b p *(λ),m 2/g)is de fined as the scattering coef ficient per unit of TSM concentration.The values of b p *(532)exhibited region-to-region variations,from 0.29m 2/g to 0.79m 2/g,with an average value of 0.58m 2/g (Table 4).The lowest b p *(532)value was found in Lake Dianchi,with strong local variation.The highest b p *(532)value was observed in Lake Taihu.The b p *(532)values in Lake Taihu (with an average b p *(532)of 0.58m 2/g in autumn and 0.7m 2/g in spring)and Chaohu (with an average b p *(532)of 0.66m 2/g)were higher than in Lake Dianchi (with an average b p *(532)of 0.37m 2/g).Scattering should be related to the ISM and OSM,which can be used to derive the average mass-speci fic scattering coef ficient for organic and inorganic particulate materials in these waters.The contribution of par-ticles to scattering can be partitioned into two parts,namely an organic and an inorganic contribution (here taking the scattering coef ficient at 532nm for example)(Snyder et al.,2008):bp 532ðÞ¼ISM bp Ã532ðÞISM þOSM bp Ã532ðÞOSMð4Þwhere b p (532)is the particle scattering coef ficient at 532nm and b p *(532)ISM (or b p *(532)OSM )is the inorganic or organic mass-speci fic particulate scattering coef ficient.Therefore,the measured particulate scattering coef ficients at 532nm were divided by the measured OSM (or ISM)amounts,and we used a linear correlation of b p (532)/OSM with ISM/OSM for our datasets to de fine the slope and intercept (that is,to derive the values of b p *(532)ISM and b p *(532)OSM )along with their standard uncertainties.Thus,the values of b p *(532)ISM and b p *(532)OSM could be derived using Eq.(4)and a linear regression method (Fig.3)(Snyder et al.,2008).As demonstrated in Fig.3,our re-sults showed that ISM (b p *(532)ISM =0.71m 2/g)has a value approxi-mately 1.6times greater than the organic suspended materials (OSM)(b p *(532)OSM =0.45m 2/g).The relationship between the mass-speci fic particulate scattering coef ficient and the TSM compositions was further investigated to deter-mine the impact of the TSM compositions on the variations in the mass-speci fic particulate scattering coef ficient in our three study areas (Fig.4).As demonstrated in Fig.4,the b p *(532)values of the three study areas completely coincided with the ratio of ISM to TSM,with the b p *(532)values increasing as the ratio of ISM to TSM increased.InFig.2.The spectral scattering coef ficients (b p )measured in Lakes Taihu (a),Chaohu (b),and Dianchi (c).Table 3Correlations between the scattering parameters and the water component concentrations for the study areas.Study areasTSM ISM OSM b p (532)Lake Taihu (summer)TSM 10.980.370.95ISM 10.160.97OSM 10.17b p (532)1Lake Taihu (spring)TSM 10.990.620.95ISM 10.510.95OSM 10.49b p (532)1Lake Chaohu TSM 10.960.630.97ISM 10.630.97OSM 10.63b p (532)1Lake Dianchi TSM 10.420.90.92ISM 1−0.020.55OSM 10.83b p (532)1Table 4Global and site-by-site statistics of the mass-speci fic (TSM)particulate scattering coef fi-cient at λ=532nm,i.e.,the b p (532)m 2/g.The maximum (Max),minimum (Min),average,and standard deviations (SD).Study areasn Max Min Average ke Taihu (autumn)560.790.30.580.1Lake Taihu (spring)310.780.430.70.1Lake Chaohu 300.70.490.660.09Lake Dianchi 250.520.290.370.04All1420.790.290.580.08311K.Shi et al./Journal of Great Lakes Research 40(2014)308–316other words,lower b p *(532)values generally were found in waters with lower organic contents.The statically signi ficant relationship (R 2=0.45,P b 0.005)between the b p *(532)values and the TSM compositions sug-gested a key role of the TSM composition in the b p *(532)variations in our study areas.We also investigated the variations of the particulate single-scattering albedo ω(λ),which was de fined as the ratio b p (λ)/c pg (λ),where b p (λ)and c pg (λ)were the scattering coef ficients and attenuation coef ficients,respectively.The ω(λ)values generally increased with wavelength (Fig.5)because the particulate absorption coef ficient var-ied with wavelength.However,there was a trough at a wavelength of approximately 675nm,which indicated a strong absorption of organic particulates at some stations.In Lake Dianchi,the trough was signi ficant at all stations because of the effects of organic particulate absorption.The values of ω(675)in Lake Dianchi were lower than in Lake Taihu and Lake Chaohu.In Lake Taihu and Lake Chaohu,the ω(675)values from most stations were between 0.97and 0.99.Spectral dependence of the particulate scattering coef ficientA power law model was used to simulate the particulate scattering spectra in all the study areas.b p (532)was used as the reference because it has a good relationship with the scattering coef ficients at the other wavelengths and can minimize errors from an overly broad wavelength interval in the regression analysis.For each station,Eq.(5)was fit to the measured b p (λ)spectrum by minimizing the weighted square sum of the difference between the modeled (Eq.(5))and measured b p (λ)values.The visible and near-IR wavelength regions were considered.The average values of the spectral slopes (γ)at Lake Taihu were 0.93and 0.82in autumn and spring,respectively.In Lake Chaohu and Lake Dianchi the mean values were 0.85and 0.47,respectively.We were able to obtain an average γvalue of 0.83throughout the study areas and dates in Lake Taihu and Lake Chaohu.Therefore,the wavelengthdependency slopes are similar to the results obtained in previous stud-ies (Morel,1988;Song and Tang,2006;Sun et al.,2009).bp λðÞ¼bp 532ðÞλ532−γð5ÞAs a result,two models,i.e.,Eq.(6)for Lake Taihu and Lake Chaohu and Eq.(7)for Lake Dianchi were obtained for simulating the particulate scattering spectra:bp λðÞ¼bp 532ðÞλ532−0:83ð6Þbp λðÞ¼bp 532ðÞλ532−0:47ð7ÞMAPE ¼1n X n i ¼1bp λðÞi −bp λðÞi 0bp λðÞi:ð8ÞTo assess the precision of the two models,the dataset was used to carry out error analysis.The mean absolute percentage errors(MAPE,Fig.3.The linear correlation of b p (532)/OSM (i.e.b p measured at 532nm divided by OSM)withISM/OSM.Fig.4.The relationship between the mass-speci fic scattering coef ficient at 532nm(b p *(532)and the ISM/TSMratio.Fig.5.The single-scattering albedo spectra (ratio,b p (λ)/c pg (λ),of scattering to attenuation coef ficients in Lakes Taihu (a),Chaohu (b),and Dianchi (c).312K.Shi et al./Journal of Great Lakes Research 40(2014)308–316Eq.(8))between the modeled and measured scattering values were cal-culated using Eq.(8).We used the scattering coef ficient at four wave-lengths (440,675,710,and 750nm)to assess the precision of the simulated power-law model (Table 5).The four wavelengths were selected because re flectance at these wavelengths is generally used for remotely estimating water quality.The model (Eq.(5))with high preci-sion was suitable for simulating the scattering coef ficients at Lake Taihu and Lake Chaohu.However,the model (Eq.(7))failed to simulate the scattering coef ficient at wavelengths where the phytoplankton absorp-tion is strong.This poor performance can be attributed to the organic particle absorption.The relative error of Eq.(7)is greater than 10%at 675nm for Lake Dianchi.Doxaran et al.(2009)discussed the effects of organic particle absorption on the scattering coef ficients and gave a new model by taking into account the particulate absorption coef ficient when simulating the spectral scattering coef ficient (Eq.(9)):b p λðÞ¼b p 532ðÞÃλ532γ−1−tanh 0:5Ãγ2h i Ãap λðÞ:ð9ÞThe value of γis estimated using Eq.(5)and scattering coef ficients in the near-IR,and a p (λ)is the particle absorption coef ficient.According to Eq.(9),we can obtain a new model (Eq.(10))for Lake Dianchi:b p λðÞ¼b p 532ðÞÃλ5320:45−1−tanh 0:5Ã0:452h i Ãap λðÞ:ð10ÞWe calculated the mean absolute percentage errors (MAPE,Eq.(8))of the two models to compare the two scattering models for Lake Dianchi.As shown in Fig.6,in the spectral range of approximately 450–606nm,the simulation error of the common power law model (Eq.(7))is lower than the model that considers the effect of particulate absorption;in the spectrum range of approximately 606–750nm,the simulation error of the common power law model (Eq.(7))is higher than that in the model (Eq.(10))that takes into account the effect of the particulate absorption.The simulation error of the common power law model (Eq.(7))has a relatively high error peak at approximately 675nm with a MAPE value of 8%because of the effect of the organic par-ticulate absorption.The model (Eq.(10))has a relatively low error at that wavelength with a MAPE of 2%.DiscussionThe size distribution of particles in lake waters is often assumed to be well described by a power law function of the particle diameter (Boss et al.,2001a;Morel,1973).For non-absorbing spherical particles with a constant refractive index,this distribution follows a power law between zero and in finite diameter.The scattering spectral slope (γ)is related to the differential slope (j)through (Astoreca et al.,2012;Babin et al.,2003;Morel,1973;Twardowski et al.,2001):j ¼γþ3:ð11ÞEq.(11)is not valid when the imaginary refractive index is signif-icantly larger than zero.To test the scattering spectral slope,γ,we obtained the scattering spectral slope γ(420–690nm)at visible wavelengths and γ(700–750nm)at near-IR wavelengths,separate-ly.The relationships between the γ(700–750nm)and γ(420–690nm)are shown in Fig.7for Lake Taihu and Lake Chaohu and in Fig.8for Lake Dianchi.In Lake Taihu and Lake Chaohu,the relation-ship between γ(700–750nm)and γ(420–690nm)is linear with a slope close to 1.The observed relative difference between the near-IR and visible scattering spectral slopes remains limited in the case of high mineral particle content.However,in Lake Dianchi,the most striking result is the signi ficant difference between the visible and near-IR scattering spectral slopes.No correlation (Fig.8)be-tween γ(700–750nm)and γ(420–690nm)was found in Lake Dianchi.Thus,we could extract the slope of the particle distribution from γ(420–750nm)in Lake Taihu and Lake Chaohu.The γis only derived from scattering coef ficients at wavelengths from 700to 750nm,where the phytoplankton absorption is weak,which could be used for estimating the particle size in Lake Dianchi.As shown in Fig.2,in Lake Taihu (both autumn and spring)and Lake Chaohu,the spectral shape of b p (λ)is spectrally dependent on λ−γ,from the visible to the near-infrared,but for Lake Dianchi,the story is quite different.The λ−γlaw is only valid for a population of particles with a high inorganic particle content (Ahn et al.,1992;Bricaud and Morel,1986;Bricaud et al.,1998;Doxaran et al.,2009;Morel,1988;Morel and Bricaud,1981).In Lake Taihu and Lake Chaohu,the particle content is dominated by inorganic particles (Fig.9).The average slopes of the particle distribution (average j:3.92in autumn Lake Taihu,3.8in spring Lake Taihu,and 3.9in Lake Chaohu)are close to 4in Lake Taihu and Lake Chaohu.This spectral scattering coef ficient is similar to that of pure minerals.Therefore,a simple power law could simulate the var-iations in the spectral scattering coef ficient with low error.However,the spectral scattering coef ficient in Lake Dianchi is obviously affected by the particulate absorption.Such scattering reduction is typical for particle populations dominated by phytoplankton (Doxaran et al.,2009).The decrease in b p (λ)at short,visible wavelengths is highly pronounced at Lake Dianchi,where a discontinuity is systematicallyTable 5The forecast precision of the scattering coef ficient spectral model (Eq.(5))at four wavelengths in Lake Taihu and Lake Chaohu but not Lake Dianchi.440nm675nm 710nm 750nm Lake Taihu (autumn)0.010.050.040.07Lake Taihu (spring)0.020.030.050.05Lake Chaohu0.020.050.040.04Fig.6.The mean relative error (MAPE)of the two models for b p (λ).Fig.7.Plot of the visible (420–690nm)scattering coef ficient slopes as a function of the near-IR (700–750nm)slopes in Lake Taihu and Lake Chaohu.313K.Shi et al./Journal of Great Lakes Research 40(2014)308–316。
清华大学本科 《水处理工程》第一篇习题集2010_106102485

《水处理工程》第一篇水和废水物化处理的原理与工艺习题集第一章绪论1.水圈的概念?指出其上界和下界。
2.试概述我国水资源的主要特点。
3.什么叫水的自然循环和社会循环?它们之间存在着怎样的矛盾?水环境保护和水处理工程的主要任务是什么?4.地下水和地面水的性质有哪些主要差别?5.水中杂质按尺寸大小可分成几类?简述各类杂质的主要来源、特点及一般去除方法。
6.简述水污染的概念和分类。
分别列举2个点污染源和面污染源。
7.简要介绍污水中主要污染物类型和对人体的危害。
8.常用的污水水质指标有哪些?选择你认为重要的解释其含义。
9.工业废水一般具有哪些特点?请列举4种工业废水的来源并简述性质。
10.试比较生活污水和工业废水的特征。
11.试讨论水资源合理利用的战略、对策与途径。
12.对于生活用水和工业用水水质主要有哪些要求?13.给水处理有哪些基本方法?其基本流程如何?14.目前我国饮用水水源的主要污染特征是什么?15.对于微污染水源,应采用什么样的饮用水处理工艺?16.对于以富营养化湖泊水为水源的饮用水处理,应采用什么样的工艺流程?17.简述废水处理的基本方法和城市污水的一般处理流程。
18.简述废水处理技术的一级、二级和三级处理。
19.试举例说明废水处理的物理法、化学法和生物法三者之间的主要区别。
20.废水处理工艺的选择应考虑哪些因素?21.试讨论饮用水处理系统和技术的发展方向。
22.试讨论城市污水处理系统和技术的发展方向。
第二章混凝1.简述胶体的动电现象、双电层与 电位。
并试用胶粒间相互作用势能曲线说明胶体稳定性原因。
2.试比较憎水胶体和亲水胶体的特点。
3.混凝过程中,压缩双电层和吸附-电中和作用有何区别?简述硫酸铝的混凝作用机理及其与水的pH值的关系。
4.概述影响混凝效果的几个因素。
5.目前我国常用的混凝剂有哪几种?各有何优缺点?今后的发展方向?6.高分子混凝剂投量过多时,为什么混凝效果反而不好?7.“助凝”的作用是什么?什么物质可以作为助凝剂?8.为什么有时需要将PAM在碱化条件下水解成HPAM?PAM水解度是何涵义?一般要求水解度为多少?9.同向絮凝和异向絮凝的差别何在?两者的凝聚速率(或碰撞速率)与哪些因素有关?10.混凝控制指标有哪几种?你认为合理的控制指标应如何确定?11.混凝过程中,G值的真正含义是什么?沿用已久的G值和GT值的数值范围存在什么缺陷?请写出机械絮凝池和水力絮凝池的G值公式。
超高速撞击波阻抗梯度材料形成的碎片云相变特性

第42卷第4期兵工学报Vol.42No.4 2021年4月ACTA ARMAMENTARII Apr.2021超高速撞击波阻抗梯度材料形成的碎片云相变特性郑克勤1,张庆明1,龙仁荣1,薛一江1,龚自正2,武强2,张品亮2,宋光明2(1.北京理工大学爆炸科学与技术国家重点实验室,北京100081; 2.北京卫星环境工程研究所,北京100094)摘要:在超高速碰撞下,波阻抗梯度材料能使弾丸的动能更多地转变为靶板材料内能,使其发生熔化、气化等相变,分散和消耗弹丸的动能,进而实现航天器对空间碎片的防护。
以钛、铝、镁3种材料组成的波阻抗梯度材料为研究对象,借助于光滑粒子流体动力学数值模拟方法,采用Til-loston状态方程和Steinberg-Guinan本构模型,给出各材料的冲击相变判据,结合速度为7.9km/s 的超高速碰撞实验结果,验证数值模拟结果的有效性。
计算结果表明:钛、铝、镁波阻抗梯度材料在受到大于4km/s速度撞击时,形成的碎片云会发生不同程度的熔化和气化;钛、铝、镁3种组分分别在受到6km/s、5km/s、4km/s速度撞击时碎片云会发生熔化,在受到8km/s、9km/s、6km/s速度撞击时碎片云会发生气化。
关键词:超高速撞击;波阻抗梯度材料;碎片云;相变中图分类号:O313.4文献标志码:A文章编号:1000-1093(2021)04-0773-08DOI:10.3969/j.issn.1000-1093.2021.04.011Phase Transition Characteristics of Debris Cloud of Ti/Al/Mg WaveImpedance Gradient Material Subjected to Hypervelocity ImpactZHENG Keqin1,ZHANG Qingming1,LONG Renrong1,XUE Yijiang1,GONG Zizheng2,WU Qiang2,ZHANG Pinliang2,SONG Guangming2(1.State Key Laboratory of Explosion Science and Technology,Beijing Institute of Technology,Beijing100081,China;2.Beijing Institute of Spacecraft Environmental Engineering,Beijing100094,China)Abstract:In hypervelocity impact,the wave impedance gradient material helps to transfer the kinetic energy into more internal energy,which causes the melting and vapor phase transition of debris cloud,and disperses and dissipates the kinetic energy of projectile,thus protecting the spacecraft from debris cloud.The wave impedance gradient material studied in this paper is made of titanium,aluminium and magnesium alloy(TAM).The smoothed particle hydrodynamics(SPH)method is used to simulate hypervelocity impact.Impact-induced phase transition criteria of various materials are given by using Tilloston equation of state and Steinberg-Guinan constitutive model.The simulated results were compared with the experimental results with impact velocity of7.9km/s.The results show that the impactgenerated debris cloud is melted and vaporized to some extent when TAM wave impedance gradient material is impacted by the velocity more than4km/s.For Ti,Al and Mg,the debris cloud is melted at the impact velocities of6km/s,5km/s and4km/s,respectively,and it is vaporized at the impact velocities of8km/s,9km/s and6km/s.收稿日期:2021-02-03基金项目:国家重点研发计划项目(2016YFC0801204);民用航天预先研究项目(D020304)作者简介:郑克勤(1992—),女,硕士研究生。
ANSYS软件英语

拉力tensile force正应力normal stress切应力shear stress静水压力hydrostatic pressure集中力concentrated force分布力distributed force线性应力应变关系linear relationship between stress andstrain弹性模量modulus of elasticity横向力lateral force transverse force轴向力axial force拉应力tensile stress压应力compressive stress平衡方程equilibrium equation静力学方程equations of static 比例极限proportional limit应力应变曲线stress-strain curve拉伸实验tensile test屈服应力yield stress静力学方程equations of static比例极限proportional limit应力应变曲线stress-strain curve拉伸实验tensile test‘屈服应力yield stress极限应力ultimate stress轴shaft梁beam纯剪切pure shear横截面积cross-sectional area挠度曲线deflection curve曲率半径radius of curvature曲率半径的倒数reciprocal of radius of curvature纵轴longitudinal axis悬臂梁cantilever beam简支梁simply supported beam微分方程differential equation惯性矩moment of inertia静矩static moment扭矩torque moment弯矩bending momentdirectory[di'rektəri,dai-]目录Preferences n.选择权[计]参数选择preference的复数Structural adj.结构的建筑的Material props[material properties]材料属性Linear['liniə]adj.线的线型的直线的线状的长度的Elastic[i'læstik]adj.有弹性的灵活的易伸缩的n.松紧带橡皮圈isotropic[,aisəu'trɔpik]adj.[物][数]各向同性的等方性的orthotropic[,ɔ:θə'trɔpik]adj.[植]直生的正交的支架桥面合一的指一种桥设计支架结构同时也是桥面或路面anisotropic[æn,aisəu'trɔpik]adj.[物]各向异性的[物]非均质的矩形截面rectangular section矩形截面梁rectangular beam单矩形截面Simple Rectangular Section圆形截面circular cross section round section工字形截面I-shaped cross sectionmodeling['mɔdəliŋ]n.[自]建模造型立体感adj.制造模型的CS(coordinate system坐标系)使用节点定义局部坐标系统,WorkPlane使用工作平面定义局部坐标系统CS的时候会有这些提示0or CART—Cartesian adj.笛卡尔哲学的笛卡尔的1or CYLIN—Cylindrical(circular or elliptical)adj.圆柱形的圆柱体的2or SPHE—Spherical(or spheroidal)adj.球形的球面的天体的3or TORO—Toroidal adj.环形的[数]超环面的n.曲面圆环Report Generator报告生成器binary['bainəri]adj.二元的二态的二进制的Entities实体Component Manager构件管理器Comp/Assembly构件、组件Everything Below所有在下面Selecetd Volumes已选择的体component[kəm'pəunənt]adj.组成的n.成分组件comp[kɔmp]assembly[ə'sembli]n.装配集会集合Status['steitəs,'stæ-]信息Global Status全局信息Graphics['ɡræfiks]绘图General['dʒenərəl]通用Parameters[pə'ræmitə]参数P—Method高次单元法LS—DYNA显示动力分析Coupled Sets耦合设置Constraint Eqns[kən'streint]约束方程Piping Module管理块Digitize Module数字化模块Reorder Module重新安排模块Master DOF主自由度Gap Conditions间隙条件DOF Constraints自由度约束Inertia Loads惯性加载Spectrum Option频谱选项Sort Module排序模块Inertia[i'nə:ʃiə]n.惯性惰性迟钝不活动spectrum['spektrəm]n.[物]光谱[电信]频谱[心]余象范围Trace Points痕迹点Fatigue Calcs疲劳运算Path Operations路径操作Load case calcs加载情况运算TimeHist Postproc时间历程后处理器Variables['vεəriəbl]变量Configuration[kən,fiɡju'reiʃən]配置Design Opt优化设计Run-Time Stats运行-时间状态Radiation Matrix辐射矩阵Layered Elements分层的单元Properties['prɔpəti]属性Specified Real Const['spesəfai,-si-]指定的实参数Layer Data层数据Specified Section Props指定截面的属性Specified Matl,All Temps指定Matl,所有温度Local Coord Sys局部坐标系Database Summary数据库摘要Initial Conditions初始条件initial[i'niʃəl]adj.最初的n.词首大写字母Joint Element DOF Constraints接缝单元自由度约束条件Array Parameters矩阵参数Pan Zoom Rotate移动缩放旋转View Settings视图设置Viewing Direction视图方向Angle of Rotation旋转角度Magnification放大倍数Focus Point焦点Rotational Center旋转中心Perspective View透视图Automatic Fit Mode自动适合模式Numbering编号Symbols符号Style风格Hidden Line Option隐藏线选项Size and Shape尺寸和外形Edge Options边缘选项Contours等值线Uniform Contours均布等值线Non-uniform Contours非均布等值线Contour Style轮廓风格Contour Labeling轮廓标签Graphs图表Viewing Control视图控制Modify Curve更改曲线Modify Grid更改网格Modify Axes更改轴Colors颜色Create Color Map创建颜色图Load Color Map加载颜色图Save Color Map保存颜色图Banded Contour Map波段等高线图Default Color Map默认颜色图Reverse Video反白显示Numbered Item Colors编号项目颜色Light Source光源Translucency[trænz'lju:sənsi,træns-,trɑ:n-]半透明Texturing['tekstʃəriŋ]材质Display Texturing显示材质Remove Volume Texturing删除体材质Display Picture Background显示图片背景Shaded Background荫背景Textured Background有织纹的背景Contour Legend轮廓图例Text Legend文本图例Floating Point Format漂浮点格式Displacement Scaling变形比例Vector Arrow Scaling矢量箭头比例Symmetry Expansion对称膨胀因子Periodic/Cyclic Symmetry周期的、循环的对称Cyclic Expansion循环的膨胀因子No Expansion无膨胀因子Font Controls字体控制Legend Font图例字体Entity Font实体字体Anno/Graph Font Anno/图表字体Window Controls窗口控制Window Layout窗口布置Window Options窗口选项Erase Options删除选项Immediate Display即时显示Animate动画Annotation[,ænəu'teiʃən]n.注释注解释文Load Bitmap File加载位图文件Device Options设备选项Redirect Plots重新定向绘图Save/Restore/Reset Plot Ctrls保存/恢复/取消绘图控制Capture/Restore Image捕获/恢复图形Write Metafile写图元文件Invert White/Black转换成白/黑色Multi-Plot Controls/layout多-绘图控制/多-窗口布局Best Quality Image最好的质量图像6.WOEKPLANE目录下Offset WP to偏移工作平面到Align WP with通过。
INTERNATIONAL CHRONOSTRATIGRAPHIC CHART

0.01170.1260.7811.802.583.6005.3337.24611.6213.8215.9720.4423.0328.133.938.041.347.856.059.261.666.072.1 ±0.283.6 ±0.286.3 ±0.589.8 ±0.393.9100.5~ 113.0~ 125.0~ 129.4~ 132.9~ 139.8~ 145.0~ 145.0152.1 ±0.9157.3 ±1.0163.5 ±1.0166.1 ±1.2168.3 ±1.3170.3 ±1.4174.1 ±1.0182.7 ±0.7190.8 ±1.0199.3 ±0.3201.3 ±0.2~ 208.5~ 227254.14 ±0.07259.8 ±0.4265.1 ±0.4268.8 ±0.5272.3 ±0.5283.5 ±0.6290.1 ±0.26303.7 ±0.1307.0 ±0.1315.2 ±0.2323.2 ±0.4330.9 ±0.2346.7 ±0.4358.9 ±0.4298.9 ±0.15295.0 ±0.18~ 237~ 242247.2251.2252.17 ±0.06 358.9 ± 0.4372.2 ±1.6382.7 ±1.6387.7 ±0.8393.3 ±1.2407.6 ±2.6410.8 ±2.8419.2 ±3.2423.0 ±2.3425.6 ±0.9427.4 ±0.5430.5 ±0.7433.4 ±0.8438.5 ±1.1440.8 ±1.2443.4 ±1.5445.2 ±1.4453.0 ±0.7458.4 ±0.9467.3 ±1.1470.0 ±1.4477.7 ±1.4485.4 ±1.9541.0 ±1.0~ 489.5~ 494~ 497~ 500.5~ 504.5~ 509~ 514~ 521~ 529~ 541.0 ±1.0~ 635850100012001400160018002050230025002800320036004000~ 4600presentSeries / Epoch Stage / Agenumerical age (Ma)E o n o t h e m / E o n E r a t h e m / E r a S y s t e m / P e r i o dSeries / Epoch Stage / Agenumerical age (Ma)E o n o t h e m / E o n E r a t h e m / E r a S y s t e m / P e r i o dSeries / Epoch Stage / Agenumerical age (Ma)System / PeriodErathem / Eranumerical age (Ma)E o n o t h e m / E o n E r a t h e m / E r a S y s t e m / P e r i o dEonothem/ Eon G S S PG S S PG S S P G S S PG S S AINTERNATIONAL CHRONOSTRATIGRAPHIC CHARTInternational Commission on StratigraphyColoring follows the Commission for theGeological Map of the World ()Chart drafted by K.M. Cohen, S.C. Finney, P.L. Gibbard(c) International Commission on Stratigraphy, February 2014To cite: Cohen, K.M., Finney, S.C., Gibbard, P .L. & Fan, J.-X. (2013; updated) The ICS International Chronostratigraphic Chart. Episodes 36: 199-204.URL: /ICSchart/ChronostratChart2014-02.pdfUnits of all ranks are in the process of being defined by Global Boundary Stratotype Section and Points (GSSP) for their lower boundaries, including those of the Archean and Proterozoic, long defined by Global Standard Stratigraphic Ages (GSSA). Charts and detailed information on ratified GSSPs are available at the website . The URL to this chart is found below. Numerical ages are subject to revision and do not define units in the Phanerozoic and the Ediacaran; only GSSPs do. For boundaries in the Phanerozoic without ratified GSSPs or without constrained numerical ages, an approximate numerical age (~) is provided.Numerical ages for all systems except Lower Pleistocene, Permian,Triassic, Cretaceous and Precambrian are taken from ‘A Geologic Time Scale 2012’ by Gradstein et al. (2012);those for the Lower Pleistocene, Permian, Triassic and Cretaceous were provided by the relevant ICS subcommissions.v 2014/02。
ASTM D4000-01

Designation:D 4000–01An American National StandardStandard Classification System forSpecifying Plastic Materials 1This standard is issued under the fixed designation D 4000;the number immediately following the designation indicates the year of original adoption or,in the case of revision,the year of last revision.A number in parentheses indicates the year of last reapproval.A superscript epsilon (e )indicates an editorial change since the last revision or reapproval.This standard has been approved for use by agencies of the Department of Defense.1.Scope *1.1This standard provides a classification system for tabu-lating the properties of unfilled,filled,and reinforced plastic materials suitable for processing into parts.N OTE 1—The classification system may serve many of the needs of industries using plastic materials.The standard is subject to revision as the need requires;therefore,the latest revision should always be used.1.2The classification system and subsequent line callout (specification)is intended to be a means of identifying plastic materials used in the fabrication of end items or parts.It is not intended for the selection of materials.Material selection should be made by those having expertise in the plastics field after careful consideration of the design and the performance required of the part,the environment to which it will be exposed,the fabrication process to be employed,the inherent properties of the material not covered in this document,and the economic factors.1.3This classification system is based on the premise that plastic materials can be arranged into broad generic families using basic properties to arrange the materials into groups,classes,and grades.A system is thus established which,together with values describing additional requirements,per-mits as complete a description as desired of the selected material.1.4In all cases where the provisions of this classification system would conflict with the referenced ASTM specification for a particular material,the latter shall take precedence.N OTE 2—When using this classification system the two-letter,three-digit suffix system applies.N OTE 3—When a material is used to fabricate a part where the requirements are too specific for a broad material callout,it is advisable for the user to consult the supplier to secure callout of the properties to suit the actual conditions to which the part is to be subjected.1.5This standard does not purport to address all of the safety concerns,if any,associated with its use.It is the responsibility of the user of this standard to establish appro-priate safety and health practices and determine the applica-bility of regulatory limitations prior to use.2.Referenced Documents 2.1ASTM Standards:D 149Test Method for Dielectric Breakdown V oltage and Dielectric Strength of Solid Electrical Insulating Materials at Commercial Power Frequencies 2D 150Test Methods for A-C Loss Characteristics and Permittivity (Dielectric Constant)of Solid Electrical Insu-lating Materials 2D 256Test Method for Determining the Izod Pendulum Impact Resistance of Notched Specimens of Plastics 3D 257Test Methods for D-C Resistance or Conductance of Insulating Materials 2D 395Test Methods for Rubber Property—Compression Set 4D 412Test Methods for Vulcanized Rubber and Thermo-plastic Rubbers and Thermoplastic Elastomers—Tension 4D 471Test Method for Rubber Property—Effect of Liq-uids 4D 495Test Method for High-V oltage,Low-Current,Dry Arc Resistance of Solid Electrical Insulation 2D 569Method for Measuring the Flow Properties of Ther-moplastic Molding Materials 5D 570Test Method for Water Absorption of Plastics 3D 573Test Method for Rubber—Deterioration in an Air Oven 4D 575Test Methods for Rubber Properties in Compression 4D 618Practice for Conditioning Plastics and Electrical Insulating Materials for Testing 3D 624Test Method for Tear Strength of Conventional Vulcanized Rubber and Thermoplastic Elastomers 4D 635Test Method for Rate of Burning and/or Extent and Time of Burning of Self-Supporting Plastics in a Horizon-tal Position 3D 638Test Method for Tensile Properties of Plastics 3D 648Test Method for Deflection Temperature of Plastics Under Flexural Load 31This classification system is under the jurisdiction of ASTM Committee D20on Plastics and is the direct responsibility of Subcommittee D20.94on Government/Industry Standardization (Section D20.94.01).Current edition approved March 10,2001.Published June 2001.Originally published as D 4000–st previous edition D 4000–00a.2Annual Book of ASTM Standards ,V ol 10.01.3Annual Book of ASTM Standards ,V ol 08.01.4Annual Book of ASTM Standards ,V ol 09.01.5Discontinued —See 1994Annual Book of ASTM Standards ,V ol 08.01.1*A Summary of Changes section appears at the end of this standard.Copyright ©ASTM International,100Barr Harbor Drive,PO Box C700,West Conshohocken,PA 19428-2959,United States.NOTICE: This standard has either been superseded and replaced by a new version or discontinued.Contact ASTM International () for the latest information.D695Test Method for Compressive Properties of Rigid Plastics3D706Specification for Cellulose Acetate Molding and Extrusion Compounds3D707Specification for Cellulose Acetate Butyrate Molding and Extrusion Compounds3D747Test Method for Apparent Bending Modulus of Plastics by Means of a Cantilever Beam3D785Test Method for Rockwell Hardness of Plastics and Electrical Insulating Materials3D787Specification for Ethyl Cellulose Molding and Extru-sion Compounds3D789Test Methods for Determination of Relative Viscos-ity,Melting Point,and Moisture Content of Polyamide (PA)3D790Test Methods for Flexural Properties of Unreinforced and Reinforced Plastics and Electrical Insulating Materi-als3D792Test Method for Density and Specific Gravity(Rela-tive Density)of Plastics by Displacement3D883Terminology Relating to Plastics3D955Test Method for Measuring Shrinkage from Mold Dimensions of Molded Plastics3D1003Test Method for Haze and Luminous Transmittance of Transparent Plastics3D1149Test Method for Rubber Deterioration—Surface Ozone Cracking in a Chamber4D1203Test Methods for V olatile Loss from Plastics Using Activated Carbon Methods3D1238Test Method for Flow Rates of Thermoplastics by Extrusion Plastometer3D1248Specification for Polyethylene Plastics Molding and Extrusion Materials3D1434Test Method for Determining Gas Permeability Characteristics of Plastic Film and Sheeting6D1435Practice for Outdoor Weathering of Plastics3D1499Practice for Filtered Open-Flame Carbon-Arc Ex-posures of Plastics3D1505Test Method for Density of Plastics by the Density-Gradient Technique3D1525Test Method for Vicat Softening Temperature of Plastics3D1562Specification for Cellulose Propionate Molding and Extrusion Compounds3D1600Terminology for Abbreviated Terms Relating to Plastics3D1693Test Method for Environmental Stress-Cracking of Ethylene Plastics3D1709Test Methods for Impact Resistance of Plastic Film by the Free-Falling Dart Method3D1784Specification for Rigid Poly(Vinyl Chloride)(PVC) Compounds and Chlorinated Poly(Vinyl Chloride) (CPVC)Compounds3D1822Test Method for Tensile-Impact Energy to Break Plastics and Electrical Insulating Materials3D1898Practice for Sampling of Plastics7D1929Test Method for Ignition Properties of Plastics3D2116Specification for FEP-Fluorocarbon Molding and Extrusion Materials3D2137Test Methods for Rubber Property—Brittleness Point of Flexible Polymers and Coated Fabrics4D2240Test Method for Rubber Property—Durometer Hardness4D2287Specification for Nonrigid Vinyl Chloride Polymer and Copolymer Molding and Extrusion Compounds3D2288Test Method for Weight Loss of Plasticizers on Heating3D2565Practice for Operating Xenon Arc-Type Light-Exposure Apparatus With and Without Water for Exposure of Plastics8D2583Test Method for Indentation Hardness of Rigid Plastics by Means of a Barcol Impressor8D2584Test Method for Ignition Loss of Cured Reinforced Resins8D2632Test Method for Rubber Property—Resilience by Vertical Rebound4D2843Test Method for Density of Smoke from the Burn-ing or Decomposition of Plastics8D2863Test Method for Measuring the Minimum Oxygen Concentration to Support Candle-Like Combustion of Plastics(Oxygen Index)8D2951Test Method for Resistance of Types III and IV Polyethylene Plastics to Thermal Stress-Cracking8D3012Test Method for Thermal Oxidative Stability of Propylene Plastics,Using a Biaxial Rotator8D3029Test Methods for Impact Resistance of Flat,Rigid Plastic Specimens by Means of a Tup(Falling Weight)9 D3294Specification for PTFE Resin Molded Sheet and Molded Basic Shapes8D3295Specification for PTFE Tubing8D3296Specification for FEP-Fluorocarbon Tube8D3350Specification for Polyethylene Plastics Pipe and Fittings Materials8D3418Test Method for Transition Temperatures of Poly-mers by Thermal Analysis8D3595Specification for Polychlorotrifluoroethylene (PCTFE)Extruded Plastic Sheet and Film8D3638Test Method for Comparative Tracking Index of Electrical Insulating Materials10D3713Test Method for Measuring Response of Solid Plastics to Ignition by a Small Flame11D3801Test Method for Measuring the Comparative Extin-guishing Characteristics of Solid Plastics in a Vertical Position8D3892Practice for Packaging/Packing of Plastics8D3895Test Method for Oxidative-Induction Time of Poly-olefins by Differential Scanning Calorimetry86Annual Book of ASTM Standards,V ol15.09.7Discontinued—See1997Annual Book of ASTM Standards,V ol08.01.8Annual Book of ASTM Standards,V ol08.02.9Discontinued—See1994Annual Book of ASTM Standards,V ol08.02.Re-placed by Test Methods D5420and D5628.10Annual Book of ASTM Standards,V ol.10.02.11Discontinued—See1999Annual Book of ASTM Standards,V ol08.02.D3915Specification for Poly(Vinyl Chloride)(PVC)and Chlorinated Poly(Vinyl Chloride)(CPVC)Compounds for Plastic Pipe and Fittings Used in Pressure Applications8 D3935Specification for Polycarbonate(PC)Unfilled and Reinforced Material8D3965Specification for Rigid Acrylonitrile-Butadiene-Styrene(ABS)Compounds for Pipe and Fittings8D3985Test Method for Oxygen Gas Transmission Rate Through Plastic Film and Sheeting Using a Coulometric Sensor6D4020Specification for Ultra-High-Molecular-Weight Polyethylene Molding and Extrusion Materials8D4066Specification for Nylon Injection and Extrusion Materials8D4067Specification for Reinforced and Filled Polyphe-nylene Sulfide Injection Molding and Extrusion Materials8 D4101Specification for Propylene Plastic Injection and Extrusion Materials8D4181Specification for Acetal(POM)Molding and Extru-sion Materials8D4203Specification for Styrene-Acrylonitrile(SAN)In-jection and Extrusion Materials8D4216Specification for Rigid Poly(Vinyl Chloride(PVC) and Related Plastic Building Products Compounds8D4329Practice for Operating Light and Water Apparatus (Fluorescent UV Condensation Type)for Exposure of Plastics12D4349Specificaton for Polyphenylene Ether(PPE)Mate-rials12D4364Practice for Performing Accelerated Outdoor Weathering of Plastics Using Concentrated Natural Sun-light12D4396Specification for Rigid Poly(Vinyl Chloride)(PVC) and Related Plastic Compounds for Nonpressure Piping Products12D4441Specification for Aqueous Dispersions of Polytet-rafluorethylene12D4474Specification for Styrenic Thermoplastic Elastomer Injection Molding and Extrusion Materials(TES)12D4507Specification for Thermoplastic Polyester(TPES) Materials13D4549Specification for Polystyrene Molding and Extru-sion Materials(PS)12D4550Specification for Thermoplastic Elastomer-Ether-Ester(TEEE)12D4617Specification for Phenolic Compounds(PF)12D4634Specification for Styrene-Maleic Anhydride Mate-rials(S/MA)12D4673Specification for Acrylonitrile-Butadiene-Styrene (ABS)Molding and Extrusion Materials12D4745Specification for Filled Compounds of Polytet-rafluoroethylene(PTFE)Molding and Extrusion Materi-als12D4812Test Method for Unnotched Cantilever Beam Im-pact Strength of Plastics12D4894Specification for Polytetrafluoroethylene(PTFE) Granular Molding and Ram Extrusion Materials12D4895Specification for Polytetrafluoroethylene(PTFE) Resins Produced from Dispersion12D4976Specification for Polyethylene Plastics Molding and Extrusion Materials12D5021Specification for Thermoplastic Elastomer–Chlori-nated Ethylene Alloy(TECEA)12D5046Specification for Fully Crosslinked Elastomeric Al-loys(FCEAs)12D5138Specification for Liquid Crystal Polymers(LCP)12 D5203Specification for Polyethylene Plastics Molding and Extrusion Materials from Recycled Post-Consumer HDPE Sources12D5279Test Method for Measuring the Dynamic Mechani-cal Properties of Plastics in Torsion12D5420Test Method for Impact Resistance of Flat,Rigid Plastic Specimen by Means of a Striker Impacted by a Falling Weight(Gardner Impact)12D5436Specification for Cast Poly(Methyl Methacrylate) Plastic Rods,Tubes,and Shapes12D5628Test Method for Impact Resistance of Flat,Rigid Plastic Specimens by Means of a Falling Dart(Tup or Falling Weight)12D5676Specification for Recycled Polystyrene Molding and Extrusion Materials12D5990Classification System for Polyketone Injection and Extrusion Materials(PK)12D6339Specification for Syndiotactic Polystyrene Molding and Extrusion(SPS)12D6358Classification System for Poly(Phenylene Sulfide) Injection Molding and Extrusion Materials Using ISO Methods12D6360Practice for Enclosed Carbon-Arc Exposures of Plastics12D6457Specification for Extruded and Compression Molded Rod and Heavy-Walled Tubing Made from Poly-tetrafluoroethylene(PTFE)12D6585Specification for Unsintered Polytetrafluoroethyl-ene(PTFE)Extruded Film or Tape12E29Practice for Using Significant Digits in Test Data to Determine Conformance with Specifications14E84Test Method for Surface Burning Characteristics of Building Materials15E96Test Methods for Water Vapor Transmission of Mate-rials16E104Practice for Maintaining Constant Relative Humidity by Means of Aqueous Solutions17E162Test Method for Surface Flammability of Materials Using a Radiant Heat Energy Source15F372Test Method for Water Vapor Transmission of Flex-ible Barrier Materials Using an Infrared Detection Tech-nique612Annual Book of ASTM Standards,V ol08.03.13Discontinued—See1998Annual Book of ASTM Standards,V ol08.03. Replaced by Specification D5927.14Annual Book of ASTM Standards,V ol14.02. 15Annual Book of ASTM Standards,V ol04.07. 16Annual Book of ASTM Standards,V ol04.06. 17Annual Book of ASTM Standards,V ol11.03.2.2Federal Standard:18Department of Transportation Federal Motor Vehicle Safety Standard No.3022.3Underwriters Laboratories:19UL94Standards for Tests for Flammability for Parts in Devices and Appliances2.4IEC and ISO Standards:20IEC 93Recommended Methods of Tests for V olume and Surface Resistivities of Electrical Insulation Materials IEC 112Recommended Method for Determining the Com-parative Tracking Index of Solid Insulation Materials Under Moist ConditionsIEC 243Recommended Methods of Test for Electrical Strength of Solid Insulating Materials at Power Frequen-ciesIEC 250Recommended Methods for the Determination of the Permittivity and Dielectric Dissipation Factor of Electrical Insulation Materials at Power,Audio,and Radio Frequencies Including Metre WavelengthsIEC 60695-11-10:Fire Hazard Testing—Part 11-10:Test Flames—50W Horizontal and Vertical Flame Tests ISO 62Plastics—Determination of Water AbsorptionISO 75-1Plastics—Determination of Temperature of De-flection Under Load—Part 1:General PrinciplesISO 75-2Plastics—Determination of Temperature of De-flection Under Load—Part 2:Plastics and EboniteISO 178Plastics—Determination of Flexural Properties of Rigid PlasticsISO 179Plastics—Determination of Charpy Impact Strength of Rigid MaterialsISO 180Plastics—Determination of Izod Impact Strength of Rigid MaterialsISO 294-4Plastics—Injection Moulding of Test Specimens of Thermoplastic Materials—Part 4:Determination of Moulding ShrinkageISO 527–1Plastics—Determination of Tensile Properties—Part 1:General PrinciplesISO 527-2Plastics—Determination of Tensile Properties—Part 2:Test Conditions for Moulding and Extrusion PlasticsISO 604Plastics—Determination of Compressive Proper-tiesISO 868Plastics—Determination of Indention Hardness by Means of a Durometer (Shore Hardness)ISO 877Plastics—Determination of Resistance to Change Upon Exposure Under Glass to DaylightISO 974Plastics—Determination of the Brittleness Tem-perature by ImpactISO 1183Plastics—Methods for Determining the Density and Relative Density of Non-Cellular PlasticsISO 2039-2Plastics—Determination of Hardness—Part 2:Rockwell HardnessISO 3795Road Vehicles,Tractors,and Machinery for Agriculture and Forestry—Determination of Burning Be-havior of Interior MaterialsISO 4577Plastics—Polypropylene and Propylene—Copolymers—Determination of Thermal Oxidative Sta-bility in Air-Oven MethodISO 4589Plastics—Determination of Flammability by Oxygen IndexISO 4607Plastics—Method of Exposure to Natural Weath-eringISO 4892Plastics—Methods of Exposure to Laboratory Light SourcesISO 4892–4Plastics—Methods of Exposure to Laboratory Light Sources—Part 4:Open-flame Carbon-arcISO 6603-1Plastics—Determination of Multiaxial Impact Behavior of Rigid Plastics—Part 1:Falling Dart Method ISO 6721-1Plastics—Determination of Dynamic Mechani-cal Properties—Part 1:General PrinciplesISO 6721-2Plastics—Determination of Dynamic Mechani-cal Properties—Part 2:Torsion-Pendulum Method ISO 11357-1Plastics—Differential Scanning Calorimetry—Part 1:General principles ISO 11357-3Plastics—Differential Scanning Calorimetry—Part 3:Determination of Temperature and Enthalpy of Melting and Crystallization18Available from Superintendent of Documents,ernment Printing Office,Washington,DC 20402.19Available from Underwriters Laboratories,Inc.,Publication Stock,333Pfingsten Rd.,Northbrook,IL 60062.20Available from American National Standards Institute,11W.42nd St.,13th Floor,New York,NY10036.TABLE1Standard Symbols for Generic Families With Referenced Standards and Cell TablesStandard Symbol Plastic Family Name ASTM A Standard Suggested Reference Cell Tables forMaterials Without an ASTM Standard BUnfilled Filled ABA acrylonitrile-butadiene-acrylate EABS acrylonitrile-butadiene-styrene D3965D4673AMMA acrylonitrile-methyl methacrylate EARP aromatic polyester(see LCP)ASA acrylonitrile-styrene-acrylate ECA cellulose acetate D706CAB cellulose acetate butyrate D707CAP cellulose acetate proprionate E DCE cellulose plastics,general E DCF cresol formaldehyde H HCMC carboxymethyl cellulose ECN cellulose nitrate E DCP cellulose propionate D1562CPE chlorinated polyethylene FCPVC chlorinated poly(vinyl chloride)D4396,D1784,D5260,D3915,D4216CS casein H HCTA cellulose triacetate E DEC ethyl cellulose D787E DE-CTFE ethylene-chlorotrifluoroethylene copolymer D3275EEA ethylene-ethyl acrylate FEMA ethylene-methacrylic acid FEP epoxy,epoxide H HEPD ethylene-propylene-dieneEPM ethylene-propylene polymer F D ETFE ethylene-tetrafluoroethylene copolymer D3159EVA ethylene-vinyl acetate FFCEA fully crosslinked elastomeric alloy D5046FEP perfluoro(ethylene-propylene)copolymer D2116FF furan formaldehyde D3296H HIPS impact polystyrene(see PS)LCP liquid crystal polymer D5138MF melamine-formaldehyde H HPA polyamide(nylon)D4066PAEK polyaryletherketone D__PAI polyamide-imide D5204G G PARA polyaryl amidePB polybutene-1FPBT poly(butylene terephthalate)(see TPES)PC polycarbonate D3935PCTFE polymonochlorotrifluoroethylene D1430,D3595PDAP poly(diallyl phthalate)H HPE polyethylene D1248,D4976,D3350,D4020,D5203PEBA polyether block amidePEEK polyetheretherketonePEI polyether-imide D5205PEO poly(ethylene oxide)D__PESV polyether sulfonePET poly(ethylene terephthalate),general(see TPES)PETG glycol modified polyethylene terephthalate comonomer(see TPES)PF phenol-formaldehyde D4617PFA perfluoro alkoxy alkane D3307PI polyimide G GPIB polyisobutylene FPK polyketone D5990PMMA Poly(methyl methacrylate)D788,D5436DPMP poly(4-methylpentene-1)FPOM polyoxymethylene(acetal)D4181POP polyphenylene oxide(see PPE)PP poly(propylene plastics)D4101PPA polyphthalamide D5336PPE polyphenylene ether D4349PPOX poly(propylene oxide)PPS poly(phenylene sulfide)D4067,D6358PPSU poly(phenyl sulfone)G GPS polystyrene D4549,D5676PSU polysulfone D6394PTFE polytetrafluoroethylene D3294,D3295,D4441,D4745,D4894,D4895,D6457,D6585PUR polyurethane F DTABLE1ContinuedStandard Symbol Plastic Family Name ASTM A Standard Suggested Reference Cell Tables forMaterials Without an ASTM Standard BUnfilled Filled PVAC poly(vinyl acetate)F D PVAL poly(vinyl alcohol)F DPVB poly(vinyl butyral)F DPVC poly(vinyl chloride)D2287F D PVDC poly(vinyl idene chloride)F D PVDF poly(vinyl idenefluoride)D3222PVF poly(vinylfluoride)F D PVFM poly(vinyl formal)F DPVK poly(vinylcarbazole)F DPVP poly(vinyl pyrrolidone)F DSAN styrene-acrylonitrile D4203SB styrene-butadiene E DSI silicone plastics G GS/MA styrene-maleic anhydride D4634SMS styrene-methylstyrene E DSPS syndiotactic polystyrene D6339TECEA thermoplastic elastomer-chlorinated ethylene alloy D5021TEEE thermoplastic elastomer,ether-ester D4550TEO thermoplastic elastomer-olefinic D5593TES thermoplastic elastomer-stryenic D4474TPE thermoplastic elastomer(see individual material)TPES thermoplastic polyester(general)D4507TPU thermoplastic polyurethane D5476UF urea-formaldehyde H HUP unsaturated polyester D__VDF vinylidenefluoride D5575A The standards listed are those in accordance with this classification.D__indicates that a standard is being developed by the subcommittee responsible.B Cell Tables A and B have been reserved for the referenced standards and will apply to unfilled andfilled materials covered in those standards.3.Terminology3.1Definitions—The definitions used in this classification system are in accordance with Terminology D883.4.Significance and Use4.1The purpose of this classification system is to provide a method of adequately identifying plastic materials in order to give industry a system that can be used universally for plastic materials.It further provides a means for specifying these materials by the use of a simple line call-out designation. 4.2This classification system was developed to permit the addition of property values for future plastics.5.Classification5.1Plastic materials shall be classified on the basis of their broad generic family.The generic family is identified by letter designations as found in Table1.These letters represent the standard abbreviations for plastics in accordance with Termi-nology D1600.N OTE4—For example:PA=polyamide(nylon).5.1.1The generic family is based on the broad chemical makeup of the base polymer.By its designation,certain inherent properties arespecified.TABLE 3Suffix Symbols and Requirements ASymbol CharacteristicAColor (unless otherwise shown by suffix,color is understood to be natural)Second letter A =does not have to match a standardB =must match standardThree-digit number 001=color and standard number on drawing002=color on drawingBFluid resistanceSecond letter A =reference fuel A,ASTM D 471,aged 70h at 2362°CB =reference fuel C,ASTM D 471,aged 70h at 2362°C C =ASTM #1oil,ASTMD 471,aged 70h at 10062°C D =IRM 902oil,ASTM D 471,aged 96h at 10062°CE =IRM 903oil,ASTM D 471,aged 70h at 10062°CF =Distilled water,ASTM D 471,aged 70h at 10062°CThree digit number is obtained from Suffix Table 1.It indicates change in hardness,tensile strength,elongation,and volume.Example:BC 132specifies that material,after aging in ASTM #1oil for 70h at 100°C,can have changed no more than 2Shore D points,5%tensile strength,15%elongation,and 5%in volume.CMelting point—softening pointSecond letter B =ASTM D 1525,load 10N,Rate A (Vicat)C =ASTMD 1525,load 10N,Rate B (Vicat)D =ASTM D 3418(Transition temperature DSC/DTA)(ISO 11357-1and 11357-3)G =ISO 306,load 10N,heating rate 50°C/h (Vicat)H =ISO 306,load 10N,heating rate 120°C/h (Vicat)I =ISO 306,load 50N,heating rate 50°C/h (Vicat)J =ISO 306,load 50N,heating rate 120°C/h (Vicat)K =ASTM D 1525,load 50N,Rate A (Vicat)L =ASTM D 1525,load 50N,Rate B (Vicat)Three-digit number =minimum value°C EElectricalSecond letter A =dielectric strength (short-time),ASTM D 149(IEC 243)Three-digit number 3factor of 0.1=kV/mm,minB =dielectric strength (step by step),ASTM D 149(IEC 243)Three-digit number 3factor of 0.1=kV/mm,minC =insulation resistance,ASTMD 257(IEC 93)Three-digit number 3factor of 1014=V ,minD =dielectric constant at 1MHz,ASTM D 150,max (IEC 250)Three-digit number 3factor of 0.1=valueE =dissipation factor at 1MHz,ASTM D 150,max (IEC 250)Three-digit number 3factor of 0.0001=valueF =arc resistance,ASTM D 495,minThree-digit number =valueG =volume resistivity,ASTM D 257(IEC 93)Three-digit number 3factor of 1014=V -cm,minH =comparative tracking index,ASTM D 3638,ac frequency,50Hz,0.1%ammonium chloride (IEC 112)Three-digit number =V,minJ =volume resistivity,ASTM D 257(IEC 93),V -cmK =surface resistivity,ASTM D 257(IEC 93),V (per square)First digit indicates:1=minimum requirement 2=maximum requirementFinal two digits indicate the exponential value of the base 10Example:EJ206specifies a maximum volume resistivity of 106V -cm FFlammabilitySecond letter A =ASTM D 635(burning rate)(IEC 60695-11-10)000=to be specified by userB =ASTM D 2863(oxygen index)(ISO 4589)Three-digit number =value %,maxC =ASTMD 1929,Procedure A (flash-ignition)TABLE 2Reinforcement-Filler A Symbols B and TolerancesSymbol MaterialToleranceC Carbon and graphite fiber-reinforced 62percentage points G Glass-reinforced62percentage pointsL Lubricants (for example,PTFE,graphite,silicone,and molybdenum disulfide)depends upon material and process—to be specified.M Mineral-reinforced62percentage pointsRCombinations of reinforcements and fillers63percentage points (based on the total reinforcements or fillers,or both)A Ash content of filled or reinforced materials may be determined using Test Method D 2584where applicable.BAdditional symbols will be added to this table asrequired.Symbol CharacteristicThree-digit number=value,°C,minD=ASTM D1929,Procedure B(self-ignition)Three-digit number=value,°C,minE=ASTM D3713000=to be specified by userF=ASTM D3801000=to be specified by userG=ASTM E162First two digits indicate minimum specimen thickness00to be specified05 3.00mm010.25mm06 6.00mm020.40mm079.00mm030.80mm0812.70mm04 1.60mm09>12.70mmThird digit indicates theflame spread115max5100max225max6150max350max7200max475max8>200H=E84000=to be specified by userJ=FMVSS302(ISO3795)000=to be specified by userK=density of smoke,ASTM D2843000=to be specified by userL=UL94(IEC60695-11-10)First digit indicates minimum specimen thicknessMolding Materials Thin Filmsmmµm0to be specified to be specified10.2525.020.4050.030.8075.04 1.60100.05 2.50125.06 3.00150.07 6.00175.0812.70200.09>12.70>200.0Second digit indicates type offlame test1=Vertical(94V)1=Horizontal(94H)3=125mmflame(94-5V)4=Vertical thin materials(94VTM)Third digit indicates theflame rating0=(94V/94VTM)0-refer to UL941=(94V/94VTM)1-refer to UL942=(94V/94VTM)2-refer to UL943=(94HB)1-burn rate<40mm/min4=(94HB)2-burn rate<75mm/min5=(94-5V)A no holes on plaques6=(94-5V)B with holes on plaques7=(94foam)1refer to UL948=(94foam)2refer to UL949=(94foam)H refer to UL94G Specific gravitySecond letter A=ASTM D792(tolerance60.02)(ISO1183Method A)B=ASTM D792(tolerance60.05)(ISO1183Method A)C=ASTM D792(tolerance60.005)(ISO1183Method A)D=ASTM D1505(tolerance60.02)E=ASTM D1505(tolerance60.05)F=ASTM D1505(tolerance60.005)H=ASTM D792/D1505(max)L=ASTM D792/D1505(min)Three-digit number3factor of0.010=requirement valueH Heat resistance,properties at temperatureSecond letter A=heat aged for70h at10062°C,ASTM D573B=heat aged for70h at15062°C,ASTM D573C=heat aged for70h at20062°C,ASTM D573Three-digit number is obtained from Suffix Table1.It indicates change in hardness,tensile strength,elongation and volume.Second letter D=tested at10062°CE=tested at12562°CF=tested at15062°CThree-digit numbers obtained from Suffix Table2.It indicates tensile strength,elongation,and tear strength.。
Static and dynamic behavior of concrete and granite in tension with damage

Static and dynamic behavior of concrete andgranite in tension with damageJ.T.Gomez a ,A.Shukla b,*,A.Sharma baCode 8232,Naval Undersea Warfare Center,Newport,RI 02841,USAbDynamic Photomechanics Laboratory,Department of Mechanical Engineering and Applied Mechanics,University of Rhode Island,92Upper College Rd.,Wales Hall,Kingston,RI 02881-0805,USAAbstractA series of dynamic and static tensile-splitting experiments were performed on concrete and granite specimens to investigate the e ect of induced damage on their tensile strength.These experiments were performed as part of a larger e ort investigating the penetration process into the two materials.The strain rate each specimen was subjected to remained constant for these experiments,while the level of induced damage was increased.Damage was induced into the specimens through repeated drop-weight impacts and quanti®ed using a statistical technique.The dynamic splitting experiments were performed using a split Hopkinson pressure bar (SHPB),while the static splitting experiments were conducted per the ASTM standard procedures D3967and C496.As part of the investigation,photoelastic dynamic tensile-splitting experiments were also performed to establish the validity of using static relations for the determination of dynamic tensile strength.The experiments showed that the static splitting strength was highly dependent on the orientation of the induced damage with regard to the applied loading;however the dynamic tensile strength decreased with increasing damage with no apparent dependency on the random damage orientation.Photoelastic experiments have shown that the mechanism of failure changes for the dynamically tested damaged specimens,reducing their de-pendence on damage orientation.Ó2001Elsevier Science Ltd.All rights reserved.1.IntroductionThis experimental study of the dynamic be-havior of concrete and granite with induced damage is a portion of a larger study into the multiple impact penetration of these materials.During the penetration process caused by multiple impacts,damage is accumulated in the region around the impact zone.The strength of cementedmaterials is a function of the inherent ¯aws present throughout the materials [1].By inducing damage into a material,the inherent ¯aws will grow in size and number,and the strength should decrease.Therefore,it becomes important to understand the e ect of damage on dynamic material strength in the study of multiple impact penetration.To study the e ect of induced damage on the strength of the G-mix Air Force concrete and Barre granite materials,whose properties are shown in Table 1,damage was induced into the specimens by repeatedly dropping a weight onto the face of each specimen.The amount of specimen damage was quanti®ed by a measure of the crack surface area created by thedamage.Theoretical and Applied Fracture Mechanics 36(2001)37±49/locate/tafmec*Corresponding author.Tel.:+1-401-874-2283;fax:+1-401-874-2950.E-mail addresses:gomezjt@ (J.T.Go-mez),shuklaa@ (A.Shukla),sharmaa@ (A.Sharma).0167-8442/01/$-see front matter Ó2001Elsevier Science Ltd.All rights reserved.PII:S 0167-8442(01)00054-4Photographs of the specimens were taken before and after the damage was induced.A grid of test lines was superimposed on the damaged specimen photograph,and the intercepts of the visible cracks with the grid lines were counted.The crack surface area per specimen volume was calculated using a statistical microscopy technique[2].The quasi-static tensile-splitting strengths of the damaged and undamaged specimens were deter-mined using ASTM standard procedures for both the concrete and granite specimens.To determine the dynamic tensile-splitting strength as a function of damage,a split Hopkin-son pressure bar(SHPB)was used to load the specimens diametrically with a dynamic stress wave.For these experiments it is assumed that the peak tensile-splitting stress of the specimen can be calculated from the peak transmitted compressive strain measured in the transmitter bar using the static relationshipfor sp litting stress,which is a function of load and specimen geometry[3].To ensure the validity of this assumption,photoelastic dynamic tensile-splitting experiments were per-formed on brittle,transparent polymer discs made of Homalite-100.The photoelastic experiments showed that the specimens were in equilibrium,i.e.the opposing contact loads were equal,for a relatively long pe-riod of time prior to failure.Also the experiments determined that once in equilibrium,the dynamic stress®eld in the specimen is identical to the stress ®eld in a statically loaded specimen.The experimental results showed that both the concrete and granite static tensile-splitting strength randomly decreased as the damage was increased, and in some cases remained unchanged in highly damaged specimens.In contrast,the dynamic ten-sile-splitting strength of the concrete and granite decreased in an orderly manner as the level of damage was increased.These results are due to the random orientation of the specimen in the loading apparatus.The static splitting strength was highly dependent on the damage orientation while the dynamic splitting strength was not.Photoelastic dynamic splitting experiments using damaged Ho-malite-100specimens determined that the failure mechanism of the damaged specimens changed, reducing their dependence on damage orientation as compared to statically tested specimens.2.Experimental procedure2.1.Quasi-static tensile-splitting experiments ASTM standard Brazilian,or tensile-splitting, experiments are usually performed on concrete or rock[4,5].For these types of materials it is di cult to fabricate and test typical``dog bone''speci-mens.The Brazilian tensile test was developed in order to indirectly determine the tensile strength of brittle materials using cylindrical specimens.Based on elasticity theory,the two-dimensional stress ®eld in the disc can be derived and then simpli®ed to examine only the stress along the loading line [6].Taking into account the specimen thickness,L, the stress distribution for the Brazilian splittingTable1Properties and composition of G-mix concrete and Barre graniteMaterial G-mix concrete Barre granite Compressive strength(MPa)41172.0Splitting tensile strength(MPa) 3.19.4Density(kg/m3)23372619 Composition Mixture for one cubic yard(kg)Mineral content(%)757.53/8-4Limestone36.5Plagioclase592.4Concrete sand31.9Quartz164.7Portland cement,type117.8Potash feldspar108.9Class``F''¯y ash8.0Biotite164.7Water 3.0Muscovite2.8Granophyre38J.T.Gomez et al./Theoretical and Applied Fracture Mechanics36(2001)37±49test is given by Eqs.(1),with parameters de®ned in Fig.1[1]:r x 2P p LD ;r y 2P p LD D 2y D Ày À1: 1 As shown,a constant tensile stress,r x ,is gener-ated along the loading line.At the contact points the elasticity solution is no longer valid due to the singularity of the loading.At these points there exists a bi-axial compressive stress of equivalent levels,which,along with the bearing blocks used to apply the load,allow the specimen to fail in tension along the loading line and not locally by compres-sion.Brittle materials,with a relatively low tensile strength compared to their compressive strength,will tend to fail in tension along the loading line.For each of the splitting tensile experiments the maximum load,P ,was used to calculate the split-ting stress,r x ,at failure using the ®rst of Eqs.(1).2.2.Split Hopkinson pressure bar for tensile-splitting In order to perform tensile splitting experiments under dynamic loading,an SHPB was used in compression.However,instead of sandwiching the specimen lengthwise,the specimen was held dia-metrically between the bars using steel bearing bars to avoid local failure due to the point load (Fig.2).To load the specimen,the incident bar is impacted with a projectile ®red from a gas gun,which creates a compressive wave traveling down the bar.At the specimen,this wave will be partially re¯ected back into the incident bar and partially transmitted into the transmitter bar.Strain gages mounted at the mid points of both bars are used to record the strain waves.Assuming one-dimen-sional propagation,and negligible attenuation of the waves,the loads on each end of the specimen can be calculated as a function of time with Eqs.(2)[7]:P 1 A b E b e i e r ;P 2 A b E b e t ;2where P 1and P 2are the loads on the incident and transmitted bar contact faces,respectively,A b is the bar cross-sectional area,E b is the Young's modulus of the bar material,and e i ,e r ,e t are the incident,re¯ected,and transmitted strain pulses shifted in time to account for the mid-bar location of the strain gages.For specimens in compression,the SHPB analysis assumes that the load on each specimen face is equal,so that the specimen is in equilib-rium.With this assumption the specimen stress/strain response can be calculated [7].However,for the dynamic splitting experiment the standard analysis to obtain the specimen stress can no longer be used.For these experiments it has been assumed that the peak tensile-splitting stress of the specimen is proportional to the peak transmitted compressive strain measured in the transmitter bar by the ®rst of Eqs.(1)[3],and that the load P is now de®ned by the second of Eqs.(2).For all these experiments,a 50.8mm diameter SHPB and 406.4mm long projectile were used.The concrete specimens wereapproximatelyJ.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±493950.8mm in diameter and 21.8mm long.The granite specimens were approximately 55.1mm in diameter and 31.8mm long.Fig.3shows an un-damaged face for both the granite and concrete specimens.2.3.Photoelastic dynamic tensile-splitting experi-mentsPhotoelastic dynamic tensile-splitting experi-ments were performed on a brittle transparent material,Homalite-100,to determine the validity of using the static relations for dynamically loaded specimens (Fig.4).For the static relation to be valid,the specimen must be in equilibrium,i.e.the opposing contact loads must be equal.In similar experiments involving dynamic wave propagation in a chain of discs,it was shown that if the loading pulse width is much longer than the disc diameter,quasi-static stress ®eld equations were valid in a region close to the contact point [8].For the SHPB dynamic loading in this study,the incident pulse length is related to the projectile length.Therefore,all the photoelastic experiments were performed on a 12.7mm SHPB using a 203mm long projectile,giving a strain pulse of approximately 406mm in length.The gas gun pressure was initially varied from 0.207to 0.414MPa to deter-mine the pressure required to obtain fracture in the specimen.The specimens for all the dynamic splitting experiments were Homalite-100discs,25.4mm in diameter and 6.4mmthick.Fig.3.Undamaged G-mix concrete and Barre granitespecimens.40J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±492.4.Induced damage application and quanti®cation The concrete and granite specimens were tested both statically and dynamically as discussed earlier with increasing levels of induced damage.To in-duce damage in the specimens,a steel weight was dropped through a vented plastic tube,onto the specimen face.Each specimen was hit with multi-ple impacts until cracks were visible in the speci-men face.The amount of specimen damage was quanti-®ed by a measure of the crack surface area created by the damage.Photographs of the specimens were taken before and after the damage was induced.A grid of test lines was superimposed on the dam-aged specimen photograph,and the intercepts of the visible cracks with the grid lines were counted.A photograph of the grid lines being used to count the intercepts is shown in Fig.5.The crack surface area per specimen volume,S v ,is proportional to the number of intercepts by S v2N L ts2 number of interceptstest line length: 3The intercepts are counted as shown in Fig.5,and the test line length can be calculated as the lengthof grid lines that lie over the circular specimen.Forexample,for a 50mm diameter specimen the sys-tem of grid lines has a length of 1.014m.For the 46intercepts shown,the damage induced in the specimen would be 90.73m 2=m 3.This method was originally developed as a microscopy technique to study the ¯aws and grain boundaries of metal samples [2].For all the experiments,static and dynamic,no particular specimen orientation of the damage to the loading line was desired.The specimens were randomly oriented between the loading contacts.3.Results and discussion 3.1.Specimen equilibriumThe photoelastic experiments determined that,by subjecting the splitting specimen to a relatively long dynamic loading pulse in the SHPB,the specimen reaches equilibrium quickly and remains in equilibrium until fracture occurs.As shown (Fig.6),the wave front enters the specimen,loading only the incident contact (0l s).Both contact points of the specimen begin to be loaded when the wave travels across the specimen (10l s).When the contact loads become equal (30l s)the specimen is in equilibrium,and the photoelastic fringe patterns appear identical to a statically loaded specimen (Fig.7).These photoelastic fringe patterns are lines of constant maximum shear stress,and can be used to determine the stress ®elds in the specimens [9].Once the specimen is in equilibrium,the stress ®eld re¯ects back and forth increasing in magni-tude,as evident by the fringe patterns becoming more dense (50l s,Fig.6).The specimen remains in equilibrium until the time of failure (93l s).With this,the static stress relationship(Eqs.(1))is valid to determine the dynamic splitting strength of a specimen loaded in a SHPB with a relatively long projectile [10].3.2.Static tensile-splitting resultsAs shown,the static splitting strength of both the concrete (Fig.8)and the granite (Fig.9)asaFig.5.Grid lines superimposed over damaged specimen with intercepts highlighted.J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±4941function of damage randomly decreases to about half its virgin strength as the damage increases.However,in some cases the material retained its full strength even though the specimen was highly damaged.This is a result of the slowly developing stress ®eld being dependent on the damage orientation with respect to the loading line.As mentioned,the tensile-splitting test develops a constant tensile stress down the loading line of the specimen.If the damage cracks were parallel to the loading line,they would greatly decrease the strength.How-ever,if the damage cracks were perpendicular to the loading line,the cracks would close under thecompression in the y -direction and not a ect the splitting strength.This dependency on random damage orientation is evident in the static splitting results.3.3.Dynamic tensile-splitting resultsAs shown,the dynamic splitting strength for both the concrete (Fig.10)and the granite (Fig.11)decrease in an orderly fashion with increasing damage.This result shows that the random ori-entation of damage cracks in the specimens does not a ect the dynamic splitting strength as it did in the staticexperiments.Fig.6.Homalite-100specimen in dynamic tensile-splitting experiment.42J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±494.Photoelastic dynamic splitting experiments with damaged specimensTo examine the di erence between the static and dynamic splitting experiments with induced damage,a series of photoelastic dynamic splitting experiments was conducted using damaged Ho-malite-100specimens.The specimens,identical to the ones used previously in the equilibrium ex-periments,had a central crack initiated by tappinga short razor blade against the face.The amount of damage the cracks represented was quanti®ed using the method outlined earlier,and found to be approximately the same for each specimen.The projectile length and ®ring pressure also remained consistent with the earlier experiments.Four ad-ditional experiments were performed,two with the central crack parallel to the loading line,one with the crack oriented at an angle of 45°to the loading line,and one with the crack perpendicular to the loading line.The dynamic splitting strength of the Homa-lite-100,at an average strain rate of 200/s,dropped from 1.5times the static strengthforFig.7.Static Homalite-100tensile-splitting fringepattern.J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±4943the undamaged specimens to0.85times with the induced damage,with no orientation depen-dence.To examine the failure mechanisms of the un-damaged and damaged specimens the high-speed photography used to capture the fringe patterns was also used to examine crack propagation and specimen failure.Fig.12shows the typical failure of an undamaged dynamic splitting specimen made of Homalite-100.As shown,the splitting cracks form just outside a dark region near the contact point at approximately93l s from the initial specimen loading.Depending on the loading level one or more of the cracks propagate across the specimen causing failure.The dark regions at the loading points were found to be in-plane cracks that formed in the area of high compression stress near the contacts.These cracks tended to curve toward the surface and not cause the speci-men to fail.The crack initiation at the incident end of the specimen agrees with the experimental and numerical work performed on concrete by Tedesco et al.[11].Examining the failure of the0°damaged specimen(Fig.13),a change in the failure mechanism is evident.The central crack is unaf-fected by the initial passing of the wave front (10l s).However as the wave re¯ects back into the specimen creating the splitting stress®eld, stress concentration at the crack tips is evident with the formation of higher-order fringes(20l s). With the increased stress at the tip,the crack grows from each end of the damage toward the contact points(30l s).Once the cracks have reached the contact point the specimen has failed. Since the dynamic loading is still being applied, additional splitting cracks form at the contacts and travel through the specimen(70l s).The failure event in this case occurs in a much shorter period of time than the undamaged case,where the cracks were just beginning to form90l s after the initial loading.The splitting experiment performed with the central crack at45°to the loading line showed a similar failure pattern(Fig.14).The compressive wave front passes through the damaged area with no real e ect(10l s)until it re¯ects from the transmitter end and the tensile stress®eld begins to form.At this point we see fringe patterns around the crack tip,indicating an increase in stress (20l s).Again the cracks propagate from either side of the damage;however in this case the cracks are shown to move toward the contact points along the highest area of tensile stress(40l s).As with the0°damaged specimen,the dynamic loading is still being applied to the specimen,so once the cracks reach the contact points,addi-tional splitting cracks form(50l s).For the last experiment,the damaged specimen was placed in the SHPB to orient its central crack perpendicular to the loading line.In this con®g-uration it would be expected that the compressive stress in the specimen would cause the crack to close,and not lower the splitting strength.This is what caused the random changes in splitting strengths observed with the statically tested specimens.However,the photoelastic experiment showed that,similar to the other pre-damaged specimens,the crack initiation site was moved from the specimen incident end to the crack tips of the induced damage.Fig.15shows the crack propagation in the specimen.The wave enters the specimen and appears una ected by the damage, even after the initial re¯ection with no formation of fringes at the crack tips(0±20l s).After the next wave re¯ection,cracks can be seen initiating at the ends of the damage(40l s)and propagat-ing toward the contact points(50±60l s).Aswith 44J.T.Gomez et al./Theoretical and Applied Fracture Mechanics36(2001)37±49the other damaged specimens,failure occurs much earlier than in the undamaged specimens,where crack initiation occurs at approximately 93l s.5.ConclusionThe static and dynamic tensile-splitting exper-iments performed determined the e ect of induced levels of damage on the splitting strength of concrete and granite specimens.The static ex-periments showed that the splitting strength is highly dependent on the orientation of the dam-age with respect to the loading line.Damage cracks perpendicular to the loading line tend to be closed by the compressive stress in that direc-tion and do not e ect the strength.Damage cracks parallel to the loading line are opened by the tensile-splitting stress and decrease the strength drastically.Therefore,by randomly ori-enting the specimens in the testing machine,some specimens that were highly damaged may still have exhibited full strength if the major portion of its damage was oriented perpendicular to the loading line.In the dynamic case,the photoelastic experi-ments determined that the specimens wereinFig.12.Crack propagation of undamaged Homalite-100specimen subjected to dynamic splitting load in SHPB.J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±4945Fig.13.Crack propagation of damaged Homalite-100specimen with 0°central crack subjected to dynamic splitting load in SHPB.46J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±49equilibrium,and the dynamic stress ®eld resem-bled the static stress ®eld.This allows the use of the static stress ®eld relation to calculate the dy-namic splitting strength.For the concrete and granite specimens,the dynamic splitting strength was shown to decrease in a regular fashion with increasing damage without a dependency on the damage orientation.Additional photoelastic experiments were per-formed with damaged specimens containing a central crack,oriented at di erent angles to the loading line,parallel,45°,and perpendicular.The dynamic splitting strength of damaged specimens at an average strain rate of 200/s,dropped from 1.5times the static strength for the undamaged specimens to 0.85times with the induced dam-age,with no orientation dependence.The high-speed images of the specimen failure showed that in all cases the crack initiation moved from the incident end of the specimen to the edges of the specimen damage.The cracks then propagated along the line of maximum tensile stress toward the contact points.Crack initiation and specimen failure in the damaged specimens were also shown to occur in approximately 50l s,where the undamaged specimens experience crack initi-ation after 93l s.In the dynamic splitting experiments,the load in the specimen increases over time as the wave re¯ects back and forth [10].Since crack initiation and failure occur in a shorter period of time with the damaged specimens,there is less time for the load to increase.The calculated splitting stress,being a function of the load recorded in the transmitter bar,is therefore lower with more in-duced damage regardless of damageorientation.Fig.14.Crack propagation of damaged Homalite-100specimen with 45°central crack subjected to dynamic splitting load in SHPB.J.T.Gomez et al./Theoretical and Applied Fracture Mechanics 36(2001)37±4947。
Static global monopoles in higher dimensional space time

Static global monopoles in higher dimensional space timeR.Mukherjee and F.RahamanDepartment of MathematicsJadavpur University, Kolkata – 700 032, IndiaE-mail: farook_rahaman@Abstract:We present an exact solution around global monopole resulting from the breaking of a global S0(3) symmetry in a five dimensional space time. We have shown that the global monopole in higher dimensional space time exerts gravitational force which is attractive in nature. It is also shown that the space around global monopole has a deficit solid angle. Finally, we study monopole in higher dimensional space time within the framework of Lyra geometry.Pacs Nos: 98.80 cq ; 04.20 jbKey words: Global monopole, Higher dimension, Lyra geometry Introduction:The idea of higher dimensional theory was originated in Super String and Super Gravity theories with the other fundamental forces in nature. To find a theory which unifies gravity with the other forces in nature remains an open problem in quantum field theory even today. Developments in Super String theories have stimulated the study of physics in higher dimensional space times [1]. More over, solutions of Einstein field equations in higher dimensional space times are believed to be physical relevance possibly at the extremely early times before the Universe underwent the compactification transitions. As a result higher dimensional theory is receiving great attention both in cosmology and particle physics. In quantum field theory, when a symmetry has broken during the phase transitions, several topological defects will arise [2]. Global monopole ( a kind of topological defect which is formed when a global symmetry is broken ) is important objects both Particle Physicists and Cosmologists which predicted to exist in Grand Unified Theory. Using a suitable scalar field it was shown that the phase transitions on the early Universe can give rise to such objects which are nothing but the topological knots in the vacuum expectation value of the scalar field and most of their energy is concentrated in a small region near the monopole core.In 1989, Barriola and Vilenkin (BV) [3] have shown an approximate solution of the Einstein equations for the metric out side a global monopole, resulting from a global S0(3) symmetry breaking. Banerjee et al [4] has extended the work of BV to higher dimension. Their space time has the topology of R1 X S1 X S2 X S1. Their five dimensional monopole metric was not unique where as BV monopole metric was unique.In this work, we would like to consider global monopole in higher dimensional space time with topology is R1 X S1 X S3. The motivation of this work is to look forward whether the global monopole shows any significant properties due to consideration of the space time with topology R1 X S1 X S3 .While attempting to unify gravitation and electromagnetism in a single space time geometry, Weyl [5] showed how one can introduce a vector field with an intrinsic geometrical significance. But this theory was not accepted as it was based on non-integrability of length transfer. Lyra [6] proposed a new modifications of Riemannian geometry by introducing a gauge function which removes the non-integrability condition of a vector under parallel transport.In consecutive investigations Sen [7] and Sen and Dunn [8] proposed a new scalar tensor theory of gravitation and constructed an analog of Einstein field equations based on Lyra’s geometry which in normal gauge may be written asR ab – ½ g ab R + 3/2 *ϕa *ϕb – ¾ g ab*ϕc *ϕc = – 8 π G T ab (1)where *φa is the displacement vector and other symbols have their usual meaning as in Riemannian geometry.According to Halford [9], the present theory predicts the same effects within observational limits, as far as the classical solar system tests are concerned, as well as tests based on the linearized form of field equations. Soleng [10] has pointed out that the constant displacement field in Lyra’s geometry will either include a creation field and be equal to Hoyle’s creation field cosmology or contain a special vacuum field which together with the gauge vector term may be considered as a cosmological term. Subsequent investigations were done by several authors in scalar tensor theory and cosmology within the frame work of Lyra geometry [11]. Recently, Rahaman et al and other authors have studied some topological defects within the framework of Lyra geometry[12].In the present work, we also derive the solutions for the higher dimensional space time metric out side a global monopole within the framework of Lyra geometry in normal gauge i.e. displacement vector *φa= ( β , 0 ,0 ,0 ,0 ) , where β is a constant .In section 3, we have studied higher dimensional global monopole in Lyra geometry. Motion of the test particle in the gravitational field of higher dimensional global monopoles are discussed in section 4. The paper ends with a short discussion in section 5.2. Global monopole in general relativity:In this section we closely follow the formalism of BV and take the Lagrangian as L = ½ ∂μΦi∂μΦi – ¼ λ (ΦiΦi – η2 ) 2 (2)where Φi is a multiplet of scalar fields, i = 1,2,3,4 (where η is the energy scale of symmetry breaking and λ is a constant).The field configuration describing a monopole is taken asΦi = η f(r) (x i / r) (3)where x i x i = r 2.[ Actually (x i / r) ≡ n i is a unit vector (n i n i = 1) in dimensional Euclidean space with components n4 = cos ψ, n3 = sin ψ cos φ, n2 = sin ψ sin φ cos θ,n2 = sin ψ sin φ sin θ ]The metric ansatz describing a monopole can be taken asds 2 = – A(r) dt 2 + B(r) dr 2 + r 2 ( dθ2 + sin2θ dφ2+ sin2θ sin2φ dψ2) (4)Using the Lagrangian (2) and metric (4) the components of energy momentum tensorscan be written via [4]T ab = 2(∂L / ∂g ab ) – L g ab (5)as follows:T t t = η2 [(f 1) 2 / 2 B] + (3/2) η2 (f 2 / r2 ) + ¼ λ (η2 f 2 – η2 )2 (6)T r r = – η2[(f 1) 2 / 2 B] + (3/2)η2 (f 2 / r2 ) + ¼ λ (η2 f 2 – η2 )2 (7)Tθθ = Tφφ = Tψψ = η2 [(f 1) 2 /2B] + ¼ λ (η2 f 2 – η2 )2 + ½ η2 (f 2 / r2 ) (8)( prime denotes the differentiation w.r.t. ‘r’ )It can be shown that in flat space the monopole core has a size δ ~ √λη–1 and mass , M core ~ λ- 1/ 2η. Thus if η < < m p where m p is the plank mass, it is evident that we can still apply the flat space approximation of δ and M core .This follows from the fact that in this case the gravity would not much influence on monopole structure.Banerjee et al assumed that f = 1 out side the monopole core [4].With this result the energy stress tensors assume the following formT t t = T r r = 3(η2 /2 r2 ) ; Tθθ = Tφφ = Tψψ = ½ (η2 / r2 ) (9)3 [B1/ (2rB2 )] – [3 / ( Br 2 ) ] + (3 / r 2 ) = 12πG(η2 / r2 ) (10)– [3/(Br 2)] + (3/r 2 ) – 3[A1/(2rAB)] = 12πG(η2 / r2 ) (11)[A1B1/4AB] – [A1 1/2AB] + [(A1) 2/(4BA2 )] + [B1/(r B2 )] –[A1/ABr] – [1/(Br 2) ] + (1/r 2 ) = ½ (η2 / r2 ) (12)Equation (10), we getZ 1 + 2(Z /r) = – 2 [( 1 – 4 πGη2 ) / r ] (13)where Z = – (1 / B)Solving this equation, we getB = [1 – 4 πGη2 – (C/ r 2 )] – 1 (14)where C is an integration constant.Now subtracting eq.(11) from eq.(12) , we get3[B1/(2 r B 2 )] + 3[A1/(2rAB)] = 0 (15)This impliesAB = 1 (16)( without any loss of generality we can take the integration constant to be unity .)Thus the solution isA = B –1 = [1 – 4 πGη2 – (C/ r 2 )] (17)Diagram of the metric coefficient g tt for 8πGη2 = 10 – 6 and different values of C . ( C = .002, for yellow line; C = .006, for red line, C = .009, for green line )Diagram of the metric coefficient g rr for 8πGη2 = 10 – 6 and different values of C . ( C = .002, for yellow line; C = .006, for red line, C = .009, for green line )It is of some interest to calculate bending of light in the above fieldin the plane θ = ½ π.The equation for the light track in the ψ = constant hyper surfaces isk2 – h2(dU/dφ)2 – h2 U2 (1 – 4 πGη2 – C U 2 ) = 0 (18)The constants k , h are defined byA (dt/dp) = k and r2 dφ/dp = h .p being the affine parameter along the light path,andr = (1 / U ) (19)From eq.(18) , one get [ writing ξ = (1 – 4 πGη2 ) ½φ ]( d2U/dξ2 ) + U [ 1 – 2CU2 (1 – 4 πGη2 ) – ½ ] = 0 (20)If the light ray does not penetrate in to the monopole core, the last term is small and one may write the above equation in the form(d2U/dξ2 ) + U = 2CU 3 (21)The approximate solution of this equation isU = U0 cos( αξ ) + U1 cos( 3αξ ) (22)with U1 = – C ( U0 2 / 16 ) < < U0 and α2 = 1 – 3(C U0 2 / 2).For U = 0 ,one gets , αξ = ± ½ πorφ = ± ½ π (1 – 4 πGη2 ) – ½ [1 – 3C (U0 2 / 2 )] -- ½ (23)And bending comes out asπ [ 2πGη2 + ¾ C U0 2 ] (24)3. Global monopole in Lyra geometry:In this section, we shall consider higher dimensional global monopole in Lyra geometry.We have taken the same energy momentum tensors as before.The field equation (1) for the metric (4) , reduces to3[B1 / (2rB 2 )] – [3 / Br 2 ] + (3/r 2 ) – ¾ (β2/A) = 12πG(η2 / r2 ) (25)– [3/(Br 2)] + (3/r 2 ) – 3[A1/(2rAB)] + ¾ (β2/A) = 12πG(η2 / r2 ) (26)[A1B1/4AB] – ½ [A11/AB] + [(A1)2/(4BA2)] + [B1/(r B2)] – [A1/ABr] – [1/(Br2)]+ 1/r2 + ¾ (β2/A) = 4πG (η2 / r2 ) (27)Now subtracting eq.(26) from eq.(25) , we get( AB ) 1 = β2 r B2 (28)Since β≠ 0, we never get the general relativity like solution . According to BV, a global monopole solution should have f = 1 as r →∞. The dependence of η2 of the asymptotic expansion of f is very weak. It appears that the asymptotic behavior of the monopolesolution is quite independence of the scale of symmetry break down up to values as largeas the planck scale [ η2 = ( 4πG ) –1 ] . However, in order to confirm the existence of monopole solutions up to η2 = ( 4πG ) –1, we have to obtain the values of A and B fromthe field equations.Adding eq.(25) with eq.(26), we get ,[ B1 / ( r B 2 )] – (1 / B ) [(A1/rA) + (4 / r2 ) ] = 4 [( 1 – 4 πGη2 ) / r 2 ] (29)Using η2 = ( 4πG ) –1, we get from eq.(29)(B1/ B) = (A1/A) + (4 / r) (30)This impliesB = B0 A r 4 (31)( here, B0 is an integration constant )Using eq.(30) , from eq.(28) , we get2(A1/A) + (4 / r) = β2 B0 r 5 (32)Solving eq.(32) , we getA = (A0/r2) exp[(1/12) β2 B0 r 6 )] (33)( here, A0 is an integration constant )Thus the higher dimensional monopole in Lyra geometry takes the following formds 2 = – (A0/r2) exp[(1/12) β2 B0 r 6 )]dt 2 + B0A0r2 exp[(1/12) β2 B0 r 6 )]dr 2 + r 2 dΩ3 2 (34)From the metric itself , it is quite apparent that there is no singularity at a finite distant from the monopole core.Diagram of the metric coefficient g tt for global monopole in Lyra geometry for different values of the displacement vector ( taking A0 = .2 , B0 = 1 and β2 = 9.6, for yellow line; β2 = 1.2, for red line, β2 = 4.8, for green line ).Diagram of the metric coefficient g rr for global monopole in Lyra geometryfor different values of the displacement vector ( taking A0 = .2 , B0 = 1 and β2 = 9.6, for yellow line; β2 = 1.2, for red line, β2 = 4.8, for green line ).4. Motion of a test particles:Let us consider a relativistic particle of mass m moving in the gravitational field of the monopole described by eq. (4).The Hamilton – Jacobi ( H-J) equation is [13]– (1/A)(∂S/∂ t)2 +(1/B)(∂S/∂r)2 +(1/r2)[(∂S/∂x1)2 +(∂S/∂x2)2+(∂S/∂x3)2] + m2 = 0 (35)with x1 , x2 , x3 are the co ordinates on the surface of the 3 – sphere .Take the ansatzS ( t , r, x1, x2 , x3 ) = – E.t + S1(r) + p1.x1+ p2.x2 + p3.x3 (36)as the solution to the H-J eq. (35).Here the constant E is identified as the energy of the particle and p1, p2, p3 are momentum of the particle along different axes on 3–sphere with p = (p12+ p2 2 + p3 2) ½ , as the resulting momentum of the particle .Now substituting (36) in (35) , we getS1(r) = ε∫ [ B{( E2/A) – (p2/r2) + m2 }] ½ dr (where ε = ± 1) (37)In H-J formalism, the path of the particle is characterized by [13](∂ S/∂ E) = constant, (∂ S/∂ p i ) = constant (i= 1,2,3 ) (38)Thus we get (taking the constants to be zero without any loss of generality),t = ε∫{(√ B)E / A}[ {(E2/A) – (p2/r2 ) + m2 }] – ½ dr (39)x i= ε∫{(√ B )p i / r2}[ {(E2/A) – (p2/r2 ) + m2 }] -½ dr (40)From (39) , we get the radial velocity as(dr/dt) = ( A/E√ B) [ {(E2/A) – (p2/r2 ) + m2 }] ½ (41)Now the turning points of the trajectory are given by ( dr/dt ) = 0 and as a consequence the potential curves are(E/m) ≡ V = [A {( p2/m2r2 ) + 1 }] ½ (42)We shall study the trajectory of the test particle for different situations:Case – I: Global monopole in general relativity:In this case the extremals of the potential curve are the solutions of the equationr2 [ (2p2/m2)( 1 – 4 πGη2 ) – 2m2C ] = 2Cp2 ( 1 + m – 2 ) (43)We note that if p2 < C m4( 1 – 4 πGη2 ) –1 , then the above radical has no real extremals. Hence there is no window in the parameter space to produce bound states and particles can not be trapped by the monopole.But this equation has at least one positive real root provided p2 > C m4 ( 1 – 4 πGη2 ) –1 . So it is possible to have bound orbit for the test particle. Thus the gravitational field of the global monopole is shown to be attractive in nature but here we have to imposed some restriction relating symmetry breaking scale η and mass and momentum of the test particle.The diagram of the potential curve with respect to radial coordinate ( taking (p2/m2) = 5, 8πGη2 = 10 – 6 and C = .006 ).Case – II: Global monopole in Lyra geometry:Here the extremals of the potential curve are the solutions of the equationβ2 B0 r 8 + (p2 m – 2 ) β2 B0 r 6 – 4r 2 – 8p2 m – 2 = 0 (44)This is an algebraic equation of even degree ( degree eight ) with negative last term.This equation has at least one real positive root. Thus bound orbit are possible in this situation. Hence the higher dimensional global monopole in Lyra geometry, always exert gravitational force which is attractive in nature.5. Discussions:At first, we state in brief, the nature of the BV’s four dimensional monopole [3].Solving the gravitational field equations and adopting some suitable scale changes,BV arrived at the metricds 2 = – dt 2 + dr 2 + (1 – 8πGη2 ) r 2 dΩ2 2 (45)From this they concluded:(a) g tt1 = 0 i.e. the acceleration vector (A r ) corresponding to the unit vector alongtime coordinate lines vanish, so the monopole exerts no gravitational force.(b) The coefficient (1 – 8πGη2 ) of r 2 dΩ2 2 indicates a deficit solid angle.For our higher dimensional monopole it is obvious that A r∝ r – 3. This shows that thegravitational force falls of as the inverse cube of the distances. We also see that the spacetime around our higher dimensional monopole has a deficit solid angle.Banerjee et al higher dimensional monopole metric is not unique but our higherdimensional monopole metric is unique.For large enough values of r, the solution (17) passes over to that given by BV.A global monopole, however, is quite consistent in Lyra geometry and we obtain theexact solutions for the space time metric in some special case. The solutions representedby eq.(34) exhibits no singularity at a finite distance from the monopole core . Thisexample is important as in general relativity all the solutions for a global monopole havea singularity for finite values of r.Our higher dimensional monopole in general relativity exerts gravitational force which isattractive in nature provided some restriction to be imposed relating symmetry breakingscale η and mass and momentum of the test particle. This is quite similar to Banerjee et almonopole [4]. But higher dimensional global monopole in Lyra geometry always exertgravitational force, which is attractive in nature. Thus we see some important differencesbetween higher dimensional global monopole in Lyra geometry with the classical result. Acknowledgments:F.R is thankful to DST , Government of India for providing financial support.We are also grateful to the anonymous referee for his valuable comments.References:1. S.Weinberg, Physics in higher dimension ( World Scientific , Singapore, !986)2. A.Vilenkin , E P S Shellard , Cosmic String and other Topological defects (1994),Camb.Univ.Press . Cambridge3. M Bariolla and A Vilenkin, Phys.Rev.Lett.63, 341 (1989)4. A Banerji,S Chaterji and A A Sen, Class.Quan.Grav. 13,3141 (1996)5. Weyl H , S. Ber. Preuss.Akad.Wiss 465 (1918)6. Lyra, G (1951), Math, Z 54,52 ;7. Sen D. K, (1957), Phys. Z 149, 3118. Sen D. K and Dunn K. A, (1971), J. Math. Phys 12, 578[For brief notes on Lyra geometry see alsoA. Beesham (1988), Aust. J. Phys. 41, 833;T. Singh and G.P.Singh, (1992) Int. J. Theor. Phys. 31,1433;F.Rahaman et al, Astrophys.Space Sci. 295, 507(2005) ]9. Halford W.D (1970), Aust. J. Phys. 23, 863.10. H.H.Soleng Gen.Rel.Grav. 19,1213,(1987)11. Bharma K. S (1974), Aust. J. Phys. 27, 541 Karade T.M and Borikar S.M,Gen Rel. Grav. 1, 431 (1978); A Beesham , Astrophys.SpaceSci.127, 189(1986); T. Singh and G.P. Singh , J. Math. Phys. 32, 2456(1991);G.P. Singh and K. Desikan , Pramana, 49, 205 (1997);F.Rahaman and J K Bera , Int.J.Mod.Phys.D10, 729 (2001);F.Rahaman et al, Astrophys.Space Sci. 281, 595(2002);S. Kotambkar, A. Pradhan , Int.J.Mod.Phys.D12, 853 (2003);Anirudh Pradhan, I. Aotemshi , Astrophys.Space Sci.288:315 (2003);D.R.K.Reddy and M.V.SubbaRao ,Astrophys.Space Sci.302,157(2006);Anirudh Pradhan, J.P. Shahi, Braz.J.Phys.36, 1227 (2006);F.Rahaman et al, gr-qc/0612042; F.Rahaman et al, gr-qc/0608082;G. Mohanty, K.L. Mahanta, B.K. Bishi , Astrophys.Space Sci.310:273 (2007);F. Rahaman et al, gr-qc/0612126; F. Rahaman et al, gr-qc/070304712. Jerzy Matyjasek , Int.J.Theor.Phys.33, 967 (1994);F. Rahaman , Int.J.Mod.Phys.D 9, 775 (2000);F. Rahaman , Int.J.Mod.Phys.D10, 579 (2001);F. Rahaman et al, Mod.Phys.Lett.A19, 2785 (2004) ( arXiv: 0704.3134[gr-qc]);F.Rahaman, Astrophys.Space Sci. 280, 337 (2002) ;F. Rahaman et al, Int.J.Mod.Phys.D 10,735 (2001);Anirudh Pradhan, I. Aotemshi , G.P. Singh , Astrophys.Space Sci.288, 315 (2003) F. Rahaman, Mod.Phys.Lett.A18, 41 (2003);F. Rahaman, Astrophys.Space Sci.283, 151 (2003);G.P. Singh et al, Int.J.Mod.Phys.D 12,853 (2003);F. Rahaman, Fizika B11, 223 (2002);F. Rahaman et al, Astrophys.Space Sci.286, 581 (2003);D.R.K. Reddy , Astrophys.Space Sci.300, 381 (2005);F. Rahaman , Nuovo Cim.B 118, 17 (2003) ( gr-qc/0612058 );F. Rahaman et al, gr-qc/0605042;D.R.K. Reddy, M.V. Subba Rao , Astrophys.Space Sci.302, 157 (2006)F. Rahaman , Nuovo Cim.B 118, 99 (2003) ( arXiv: 0704.1340[gr-qc]);F. Rahaman et al, arXiv: 0705.0221[gr-qc]13. S Chakraborty, Gen.Rel.Grav. 28,1115(1996);S. Chakraborty et al , Pramana 51,689(1998)11。
弹性力学专业英语英汉互译词汇

弹性力学elasticity弹性理论theory of elasticity 均匀应力状态homogeneous state of stress 应力不变量stress invariant应变不变量strain invariant应变椭球strain ellipsoid 均匀应变状态homogeneous state ofstrain 应变协调方程equation of straincompatibility 拉梅常量Lame constants 各向同性弹性isotropic elasticity 旋转圆盘rotating circular disk 楔wedge开尔文问题Kelvin problem 布西内斯克问题Boussinesq problem艾里应力函数Airy stress function克罗索夫--穆斯赫利什维Kolosoff-利法Muskhelishvili method 基尔霍夫假设Kirchhoff hypothesis 板Plate矩形板Rectangular plate圆板Circular plate环板Annular plate波纹板Corrugated plate加劲板Stiffened plate,reinforcedPlate 中厚板Plate of moderate thickness 弯[曲]应力函数Stress function of bending 壳Shell扁壳Shallow shell旋转壳Revolutionary shell球壳Spherical shell [圆]柱壳Cylindrical shell 锥壳Conical shell环壳Toroidal shell封闭壳Closed shell波纹壳Corrugated shell扭[转]应力函数Stress function of torsion 翘曲函数Warping function半逆解法semi-inverse method瑞利--里茨法Rayleigh-Ritz method 松弛法Relaxation method莱维法Levy method松弛Relaxation 量纲分析Dimensional analysis自相似[性] self-similarity 影响面Influence surface接触应力Contact stress赫兹理论Hertz theory协调接触Conforming contact滑动接触Sliding contact滚动接触Rolling contact压入Indentation各向异性弹性Anisotropic elasticity 颗粒材料Granular material散体力学Mechanics of granular media 热弹性Thermoelasticity超弹性Hyperelasticity粘弹性Viscoelasticity对应原理Correspondence principle 褶皱Wrinkle塑性全量理论Total theory of plasticity 滑动Sliding微滑Microslip粗糙度Roughness非线性弹性Nonlinear elasticity 大挠度Large deflection突弹跳变snap-through有限变形Finite deformation格林应变Green strain阿尔曼西应变Almansi strain弹性动力学Dynamic elasticity运动方程Equation of motion准静态的Quasi-static气动弹性Aeroelasticity水弹性Hydroelasticity颤振Flutter弹性波Elastic wave简单波Simple wave柱面波Cylindrical wave水平剪切波Horizontal shear wave竖直剪切波Vertical shear wave 体波body wave无旋波Irrotational wave畸变波Distortion wave膨胀波Dilatation wave瑞利波Rayleigh wave等容波Equivoluminal wave勒夫波Love wave界面波Interfacial wave边缘效应edge effect塑性力学Plasticity可成形性Formability金属成形Metal forming耐撞性Crashworthiness结构抗撞毁性Structural crashworthiness 拉拔Drawing 破坏机构Collapse mechanism 回弹Springback挤压Extrusion冲压Stamping穿透Perforation层裂Spalling 塑性理论Theory of plasticity安定[性]理论Shake-down theory运动安定定理kinematic shake-down theorem静力安定定理Static shake-down theorem 率相关理论rate dependent theorem 载荷因子load factor加载准则Loading criterion加载函数Loading function加载面Loading surface塑性加载Plastic loading塑性加载波Plastic loading wave 简单加载Simple loading比例加载Proportional loading 卸载Unloading卸载波Unloading wave冲击载荷Impulsive load阶跃载荷step load脉冲载荷pulse load极限载荷limit load中性变载nentral loading拉抻失稳instability in tension 加速度波acceleration wave本构方程constitutive equation 完全解complete solution名义应力nominal stress过应力over-stress真应力true stress等效应力equivalent stress流动应力flow stress应力间断stress discontinuity应力空间stress space主应力空间principal stress space静水应力状态hydrostatic state of stress 对数应变logarithmic strain工程应变engineering strain等效应变equivalent strain应变局部化strain localization 应变率strain rate应变率敏感性strain rate sensitivity 应变空间strain space有限应变finite strain塑性应变增量plastic strain increment 累积塑性应变accumulated plastic strain 永久变形permanent deformation内变量internal variable应变软化strain-softening理想刚塑性材料rigid-perfectly plasticMaterial刚塑性材料rigid-plastic material理想塑性材料perfectl plastic material 材料稳定性stability of material应变偏张量deviatoric tensor of strain 应力偏张量deviatori tensor of stress 应变球张量spherical tensor of strain 应力球张量spherical tensor of stress 路径相关性path-dependency线性强化linear strain-hardening应变强化strain-hardening随动强化kinematic hardening各向同性强化isotropic hardening 强化模量strain-hardening modulus幂强化power hardening塑性极限弯矩plastic limit bendingMoment塑性极限扭矩plastic limit torque弹塑性弯曲elastic-plastic bending弹塑性交界面elastic-plastic interface 弹塑性扭转elastic-plastic torsion 粘塑性Viscoplasticity非弹性Inelasticity理想弹塑性材料elastic-perfectly plasticMaterial 极限分析limit analysis极限设计limit design极限面limit surface上限定理upper bound theorem上屈服点upper yield point下限定理lower bound theorem下屈服点lower yield point界限定理bound theorem初始屈服面initial yield surface后继屈服面subsequent yield surface屈服面[的]外凸性convexity of yield surface 截面形状因子shape factor of cross-section沙堆比拟sand heap analogy 屈服Yield 屈服条件yield condition屈服准则yield criterion屈服函数yield function屈服面yield surface塑性势plastic potential 能量吸收装置energy absorbing device 能量耗散率energy absorbing device 塑性动力学dynamic plasticity 塑性动力屈曲dynamic plastic buckling 塑性动力响应dynamic plastic response 塑性波plastic wave 运动容许场kinematically admissibleField 静力容许场statically admissibleField 流动法则flow rule速度间断velocity discontinuity滑移线slip-lines滑移线场slip-lines field移行塑性铰travelling plastic hinge 塑性增量理论incremental theory ofPlasticity 米泽斯屈服准则Mises yield criterion 普朗特--罗伊斯关系prandtl- Reuss relation 特雷斯卡屈服准则Tresca yield criterion洛德应力参数Lode stress parameter莱维--米泽斯关系Levy-Mises relation亨基应力方程Hencky stress equation赫艾--韦斯特加德应力空Haigh-Westergaard 间stress space洛德应变参数Lode strain parameter德鲁克公设Drucker postulate盖林格速度方程Geiringer velocityEquation结构力学structural mechanics结构分析structural analysis结构动力学structural dynamics拱Arch三铰拱three-hinged arch抛物线拱parabolic arch圆拱circular arch穹顶Dome空间结构space structure空间桁架space truss雪载[荷] snow load风载[荷] wind load土压力earth pressure地震载荷earthquake loading弹簧支座spring support支座位移support displacement支座沉降support settlement超静定次数degree of indeterminacy机动分析kinematic analysis结点法method of joints截面法method of sections结点力joint forces共轭位移conjugate displacement影响线influence line 三弯矩方程three-moment equation单位虚力unit virtual force刚度系数stiffness coefficient柔度系数flexibility coefficient力矩分配moment distribution力矩分配法moment distribution method 力矩再分配moment redistribution分配系数distribution factor矩阵位移法matri displacement method 单元刚度矩阵element stiffness matrix 单元应变矩阵element strain matrix 总体坐标global coordinates贝蒂定理Betti theorem高斯--若尔当消去法Gauss-Jordan eliminationMethod 屈曲模态buckling mode 复合材料力学mechanics of composites 复合材料composite material 纤维复合材料fibrous composite单向复合材料unidirectional composite泡沫复合材料foamed composite颗粒复合材料particulate composite 层板Laminate夹层板sandwich panel正交层板cross-ply laminate 斜交层板angle-ply laminate 层片Ply 多胞固体cellular solid 膨胀Expansion压实Debulk劣化Degradation脱层Delamination脱粘Debond 纤维应力fiber stress层应力ply stress层应变ply strain层间应力interlaminar stress比强度specific strength强度折减系数strength reduction factor 强度应力比strength -stress ratio 横向剪切模量transverse shear modulus 横观各向同性transverse isotropy正交各向异Orthotropy剪滞分析shear lag analysis短纤维chopped fiber长纤维continuous fiber纤维方向fiber direction纤维断裂fiber break纤维拔脱fiber pull-out纤维增强fiber reinforcement致密化Densification最小重量设计optimum weight design网格分析法netting analysis 混合律rule of mixture失效准则failure criterion蔡--吴失效准则Tsai-W u failure criterion 达格代尔模型Dugdale model 断裂力学fracture mechanics概率断裂力学probabilistic fractureMechanics格里菲思理论Griffith theory线弹性断裂力学linear elastic fracturemechanics, LEFM弹塑性断裂力学elastic-plastic fracturemecha-nics, EPFM 断裂Fracture 脆性断裂brittle fracture解理断裂cleavage fracture蠕变断裂creep fracture延性断裂ductile fracture晶间断裂inter-granular fracture 准解理断裂quasi-cleavage fracture 穿晶断裂trans-granular fracture 裂纹Crack裂缝Flaw缺陷Defect割缝Slit微裂纹Microcrack折裂Kink 椭圆裂纹elliptical crack深埋裂纹embedded crack[钱]币状裂纹penny-shape crack 预制裂纹Precrack短裂纹short crack表面裂纹surface crack裂纹钝化crack blunting裂纹分叉crack branching裂纹闭合crack closure裂纹前缘crack front裂纹嘴crack mouth裂纹张开角crack opening angle,COA 裂纹张开位移crack opening displacement,COD 裂纹阻力crack resistance裂纹面crack surface裂纹尖端crack tip裂尖张角crack tip opening angle,CTOA裂尖张开位移crack tip openingdisplacement, CTOD裂尖奇异场crack tip singularityField裂纹扩展速率crack growth rate稳定裂纹扩展stable crack growth定常裂纹扩展steady crack growth亚临界裂纹扩展subcritical crack growth 裂纹[扩展]减速crack retardation 止裂crack arrest 止裂韧度arrest toughness断裂类型fracture mode滑开型sliding mode张开型opening mode撕开型tearing mode复合型mixed mode撕裂Tearing 撕裂模量tearing modulus断裂准则fracture criterionJ积分J-integral J阻力曲线J-resistance curve断裂韧度fracture toughness应力强度因子stress intensity factor HRR场Hutchinson-Rice-RosengrenField 守恒积分conservation integral 有效应力张量effective stress tensor 应变能密度strain energy density 能量释放率energy release rate 内聚区cohesive zone塑性区plastic zone张拉区stretched zone热影响区heat affected zone, HAZ 延脆转变温度brittle-ductile transitiontempe- rature 剪切带shear band剪切唇shear lip无损检测non-destructive inspection 双边缺口试件double edge notchedspecimen, DEN specimen 单边缺口试件single edge notchedspecimen, SEN specimen 三点弯曲试件three point bendingspecimen, TPB specimen 中心裂纹拉伸试件center cracked tensionspecimen, CCT specimen 中心裂纹板试件center cracked panelspecimen, CCP specimen 紧凑拉伸试件compact tension specimen,CT specimen 大范围屈服large scale yielding小范围攻屈服small scale yielding 韦布尔分布Weibull distribution 帕里斯公式paris formula 空穴化Cavitation应力腐蚀stress corrosion概率风险判定probabilistic riskassessment, PRA 损伤力学damage mechanics 损伤Damage连续介质损伤力学continuum damage mechanics 细观损伤力学microscopic damage mechanics 累积损伤accumulated damage脆性损伤brittle damage延性损伤ductile damage宏观损伤macroscopic damage细观损伤microscopic damage微观损伤microscopic damage损伤准则damage criterion损伤演化方程damage evolution equation 损伤软化damage softening损伤强化damage strengthening损伤张量damage tensor损伤阈值damage threshold损伤变量damage variable损伤矢量damage vector损伤区damage zone疲劳Fatigue 低周疲劳low cycle fatigue应力疲劳stress fatigue随机疲劳random fatigue蠕变疲劳creep fatigue腐蚀疲劳corrosion fatigue疲劳损伤fatigue damage疲劳失效fatigue failure 疲劳断裂fatigue fracture 疲劳裂纹fatigue crack 疲劳寿命fatigue life疲劳破坏fatigue rupture 疲劳强度fatigue strength 疲劳辉纹fatigue striations 疲劳阈值fatigue threshold 交变载荷alternating load 交变应力alternating stress 应力幅值stress amplitude 应变疲劳strain fatigue 应力循环stress cycle应力比stress ratio安全寿命safe life过载效应overloading effect 循环硬化cyclic hardening 循环软化cyclic softening 环境效应environmental effect 裂纹片crack gage裂纹扩展crack growth, crackPropagation 裂纹萌生crack initiation 循环比cycle ratio实验应力分析experimental stressAnalysis工作[应变]片active[strain] gage基底材料backing material应力计stress gage 零[点]飘移zero shift, zero drift 应变测量strain measurement应变计strain gage 应变指示器strain indicator 应变花strain rosette 应变灵敏度strain sensitivity机械式应变仪mechanical strain gage 直角应变花rectangular rosette 引伸仪Extensometer应变遥测telemetering of strain 横向灵敏系数transverse gage factor 横向灵敏度transverse sensitivity 焊接式应变计weldable strain gage 平衡电桥balanced bridge粘贴式应变计bonded strain gage粘贴箔式应变计bonded foiled gage粘贴丝式应变计bonded wire gage 桥路平衡bridge balancing电容应变计capacitance strain gage 补偿片compensation technique 补偿技术compensation technique 基准电桥reference bridge电阻应变计resistance strain gage 温度自补偿应变计self-temperaturecompensating gage半导体应变计semiconductor strainGage 集流器slip ring应变放大镜strain amplifier疲劳寿命计fatigue life gage电感应变计inductance [strain] gage 光[测]力学Photomechanics 光弹性Photoelasticity光塑性Photoplasticity杨氏条纹Young fringe双折射效应birefrigent effect 等位移线contour of equalDisplacement 暗条纹dark fringe条纹倍增fringe multiplication 干涉条纹interference fringe 等差线Isochromatic等倾线Isoclinic等和线isopachic应力光学定律stress- optic law主应力迹线Isostatic 亮条纹light fringe光程差optical path difference 热光弹性photo-thermo -elasticity 光弹性贴片法photoelastic coatingMethod光弹性夹片法photoelastic sandwichMethod动态光弹性dynamic photo-elasticity 空间滤波spatial filtering空间频率spatial frequency起偏镜Polarizer反射式光弹性仪reflection polariscope残余双折射效应residual birefringentEffect应变条纹值strain fringe value应变光学灵敏度strain-optic sensitivity 应力冻结效应stress freezing effect应力条纹值stress fringe value应力光图stress-optic pattern暂时双折射效应temporary birefringentEffect脉冲全息法pulsed holography透射式光弹性仪transmission polariscope 实时全息干涉法real-time holographicinterfero - metry 网格法grid method全息光弹性法holo-photoelasticity 全息图Hologram全息照相Holograph全息干涉法holographic interferometry 全息云纹法holographic moire technique 全息术Holography全场分析法whole-field analysis散斑干涉法speckle interferometry 散斑Speckle错位散斑干涉法speckle-shearinginterferometry, shearography 散斑图Specklegram白光散斑法white-light speckle method 云纹干涉法moire interferometry [叠栅]云纹moire fringe[叠栅]云纹法moire method 云纹图moire pattern 离面云纹法off-plane moire method 参考栅reference grating试件栅specimen grating分析栅analyzer grating面内云纹法in-plane moire method脆性涂层法brittle-coating method 条带法strip coating method坐标变换transformation ofCoordinates计算结构力学computational structuralmecha-nics加权残量法weighted residual method 有限差分法finite difference method 有限[单]元法finite element method 配点法point collocation里茨法Ritz method广义变分原理generalized variationalPrinciple 最小二乘法least square method胡[海昌]一鹫津原理Hu-Washizu principle赫林格-赖斯纳原理Hellinger-ReissnerPrinciple 修正变分原理modified variationalPrinciple 约束变分原理constrained variationalPrinciple 混合法mixed method杂交法hybrid method边界解法boundary solution method 有限条法finite strip method半解析法semi-analytical method协调元conforming element非协调元non-conforming element混合元mixed element杂交元hybrid element边界元boundary element 强迫边界条件forced boundary condition 自然边界条件natural boundary condition 离散化Discretization离散系统discrete system连续问题continuous problem广义位移generalized displacement 广义载荷generalized load广义应变generalized strain广义应力generalized stress界面变量interface variable 节点node, nodal point[单]元Element角节点corner node边节点mid-side node内节点internal node无节点变量nodeless variable 杆元bar element桁架杆元truss element 梁元beam element二维元two-dimensional element 一维元one-dimensional element 三维元three-dimensional element 轴对称元axisymmetric element 板元plate element壳元shell element厚板元thick plate element三角形元triangular element四边形元quadrilateral element 四面体元tetrahedral element 曲线元curved element二次元quadratic element线性元linear element三次元cubic element四次元quartic element等参[数]元isoparametric element超参数元super-parametric element 亚参数元sub-parametric element节点数可变元variable-number-node element 拉格朗日元Lagrange element拉格朗日族Lagrange family巧凑边点元serendipity element巧凑边点族serendipity family 无限元infinite element单元分析element analysis单元特性element characteristics 刚度矩阵stiffness matrix几何矩阵geometric matrix等效节点力equivalent nodal force 节点位移nodal displacement节点载荷nodal load位移矢量displacement vector载荷矢量load vector质量矩阵mass matrix集总质量矩阵lumped mass matrix相容质量矩阵consistent mass matrix 阻尼矩阵damping matrix瑞利阻尼Rayleigh damping刚度矩阵的组集assembly of stiffnessMatrices载荷矢量的组集consistent mass matrix质量矩阵的组集assembly of mass matrices 单元的组集assembly of elements局部坐标系local coordinate system局部坐标local coordinate面积坐标area coordinates体积坐标volume coordinates曲线坐标curvilinear coordinates 静凝聚static condensation合同变换contragradient transformation 形状函数shape function试探函数trial function检验函数test function权函数weight function样条函数spline function代用函数substitute function降阶积分reduced integration零能模式zero-energy modeP收敛p-convergenceH收敛h-convergence掺混插值blended interpolation等参数映射isoparametric mapping双线性插值bilinear interpolation小块检验patch test非协调模式incompatible mode 节点号node number单元号element number带宽band width带状矩阵banded matrix变带状矩阵profile matrix带宽最小化minimization of band width 波前法frontal method子空间迭代法subspace iteration method 行列式搜索法determinant search method 逐步法step-by-step method 纽马克法Newmark威尔逊法Wilson拟牛顿法quasi-Newton method牛顿-拉弗森法Newton-Raphson method 增量法incremental method初应变initial strain初应力initial stress切线刚度矩阵tangent stiffness matrix 割线刚度矩阵secant stiffness matrix 模态叠加法mode superposition method 平衡迭代equilibrium iteration子结构Substructure子结构法substructure technique 超单元super-element网格生成mesh generation结构分析程序structural analysis program 前处理pre-processing后处理post-processing网格细化mesh refinement应力光顺stress smoothing组合结构composite structure。
弹性力学专业英语英汉互译词汇

弹性力学elasticity弹性理论theory of elasticity均匀应力状态homogeneous state of stress 应力不变量stress invariant应变不变量strain invariant应变椭球strain ellipsoid均匀应变状态homogeneous state ofstrain 应变协调方程equation of straincompatibility 拉梅常量Lame constants各向同性弹性isotropic elasticity旋转圆盘rotating circular disk 楔wedge开尔文问题Kelvin problem 布西内斯克问题Boussinesq problem艾里应力函数Airy stress function克罗索夫--穆斯赫利什维Kolosoff-利法Muskhelishvili method 基尔霍夫假设Kirchhoff hypothesis 板Plate矩形板Rectangular plate圆板Circular plate环板Annular plate波纹板Corrugated plate加劲板Stiffened plate,reinforcedPlate 中厚板Plate of moderate thickness 弯[曲]应力函数Stress function of bending 壳Shell扁壳Shallow shell旋转壳Revolutionary shell球壳Spherical shell [圆]柱壳Cylindrical shell 锥壳Conical shell环壳Toroidal shell封闭壳Closed shell波纹壳Corrugated shell扭[转]应力函数Stress function of torsion 翘曲函数Warping function半逆解法semi-inverse method瑞利--里茨法Rayleigh-Ritz method 松弛法Relaxation method莱维法Levy method松弛Relaxation 量纲分析Dimensional analysis 自相似[性] self-similarity影响面Influence surface接触应力Contact stress赫兹理论Hertz theory协调接触Conforming contact滑动接触Sliding contact滚动接触Rolling contact压入Indentation各向异性弹性Anisotropic elasticity颗粒材料Granular material散体力学Mechanics of granular media 热弹性Thermoelasticity超弹性Hyperelasticity粘弹性Viscoelasticity对应原理Correspondence principle 褶皱Wrinkle塑性全量理论Total theory of plasticity 滑动Sliding微滑Microslip粗糙度Roughness非线性弹性Nonlinear elasticity大挠度Large deflection突弹跳变snap-through有限变形Finite deformation格林应变Green strain阿尔曼西应变Almansi strain弹性动力学Dynamic elasticity运动方程Equation of motion准静态的Quasi-static气动弹性Aeroelasticity水弹性Hydroelasticity颤振Flutter弹性波Elastic wave简单波Simple wave柱面波Cylindrical wave水平剪切波Horizontal shear wave竖直剪切波Vertical shear wave 体波body wave无旋波Irrotational wave畸变波Distortion wave膨胀波Dilatation wave瑞利波Rayleigh wave等容波Equivoluminal wave勒夫波Love wave界面波Interfacial wave边缘效应edge effect塑性力学Plasticity可成形性Formability金属成形Metal forming耐撞性Crashworthiness结构抗撞毁性Structural crashworthiness 拉拔Drawing破坏机构Collapse mechanism 回弹Springback挤压Extrusion冲压Stamping穿透Perforation层裂Spalling塑性理论Theory of plasticity安定[性]理论Shake-down theory运动安定定理kinematic shake-down theorem静力安定定理Static shake-down theorem 率相关理论rate dependent theorem 载荷因子load factor加载准则Loading criterion加载函数Loading function加载面Loading surface塑性加载Plastic loading塑性加载波Plastic loading wave简单加载Simple loading比例加载Proportional loading 卸载Unloading卸载波Unloading wave冲击载荷Impulsive load阶跃载荷step load脉冲载荷pulse load极限载荷limit load中性变载nentral loading拉抻失稳instability in tension 加速度波acceleration wave本构方程constitutive equation 完全解complete solution名义应力nominal stress过应力over-stress真应力true stress等效应力equivalent stress流动应力flow stress应力间断stress discontinuity应力空间stress space主应力空间principal stress space静水应力状态hydrostatic state of stress 对数应变logarithmic strain工程应变engineering strain等效应变equivalent strain应变局部化strain localization应变率strain rate应变率敏感性strain rate sensitivity 应变空间strain space有限应变finite strain塑性应变增量plastic strain increment 累积塑性应变accumulated plastic strain 永久变形permanent deformation内变量internal variable应变软化strain-softening理想刚塑性材料rigid-perfectly plasticMaterial 刚塑性材料rigid-plastic material理想塑性材料perfectl plastic material 材料稳定性stability of material 应变偏张量deviatoric tensor of strain 应力偏张量deviatori tensor of stress 应变球张量spherical tensor of strain 应力球张量spherical tensor of stress 路径相关性path-dependency线性强化linear strain-hardening应变强化strain-hardening随动强化kinematic hardening各向同性强化isotropic hardening强化模量strain-hardening modulus幂强化power hardening 塑性极限弯矩plastic limit bendingMoment 塑性极限扭矩plastic limit torque弹塑性弯曲elastic-plastic bending 弹塑性交界面elastic-plastic interface 弹塑性扭转elastic-plastic torsion粘塑性Viscoplasticity非弹性Inelasticity理想弹塑性材料elastic-perfectly plasticMaterial 极限分析limit analysis极限设计limit design极限面limit surface上限定理upper bound theorem上屈服点upper yield point下限定理lower bound theorem下屈服点lower yield point界限定理bound theorem初始屈服面initial yield surface后继屈服面subsequent yield surface屈服面[的]外凸性convexity of yield surface 截面形状因子shape factor of cross-section沙堆比拟sand heap analogy 屈服Yield 屈服条件yield condition屈服准则yield criterion屈服函数yield function屈服面yield surface塑性势plastic potential 能量吸收装置energy absorbing device 能量耗散率energy absorbing device 塑性动力学dynamic plasticity 塑性动力屈曲dynamic plastic buckling 塑性动力响应dynamic plastic response 塑性波plastic wave运动容许场kinematically admissibleField 静力容许场statically admissibleField 流动法则flow rule速度间断velocity discontinuity滑移线slip-lines滑移线场slip-lines field移行塑性铰travelling plastic hinge 塑性增量理论incremental theory ofPlasticity米泽斯屈服准则Mises yield criterion 普朗特--罗伊斯关系prandtl- Reuss relation 特雷斯卡屈服准则Tresca yield criterion洛德应力参数Lode stress parameter莱维--米泽斯关系Levy-Mises relation亨基应力方程Hencky stress equation赫艾--韦斯特加德应力空Haigh-Westergaard 间stress space洛德应变参数Lode strain parameter德鲁克公设Drucker postulate盖林格速度方程Geiringer velocityEquation结构力学structural mechanics结构分析structural analysis结构动力学structural dynamics拱Arch三铰拱three-hinged arch抛物线拱parabolic arch圆拱circular arch穹顶Dome空间结构space structure空间桁架space truss雪载[荷] snow load风载[荷] wind load土压力earth pressure地震载荷earthquake loading弹簧支座spring support支座位移support displacement支座沉降support settlement超静定次数degree of indeterminacy机动分析kinematic analysis结点法method of joints截面法method of sections结点力joint forces共轭位移conjugate displacement影响线influence line三弯矩方程three-moment equation单位虚力unit virtual force刚度系数stiffness coefficient柔度系数flexibility coefficient力矩分配moment distribution力矩分配法moment distribution method 力矩再分配moment redistribution分配系数distribution factor矩阵位移法matri displacement method 单元刚度矩阵element stiffness matrix 单元应变矩阵element strain matrix总体坐标global coordinates贝蒂定理Betti theorem高斯--若尔当消去法Gauss-Jordan eliminationMethod 屈曲模态buckling mode复合材料力学mechanics of composites复合材料composite material 纤维复合材料fibrous composite单向复合材料unidirectional composite泡沫复合材料foamed composite颗粒复合材料particulate composite 层板Laminate夹层板sandwich panel正交层板cross-ply laminate斜交层板angle-ply laminate 层片Ply多胞固体cellular solid 膨胀Expansion压实Debulk劣化Degradation脱层Delamination脱粘Debond纤维应力fiber stress层应力ply stress层应变ply strain层间应力interlaminar stress比强度specific strength强度折减系数strength reduction factor 强度应力比strength -stress ratio 横向剪切模量transverse shear modulus 横观各向同性transverse isotropy正交各向异Orthotropy剪滞分析shear lag analysis短纤维chopped fiber长纤维continuous fiber纤维方向fiber direction纤维断裂fiber break纤维拔脱fiber pull-out纤维增强fiber reinforcement致密化Densification最小重量设计optimum weight design 网格分析法netting analysis混合律rule of mixture失效准则failure criterion蔡--吴失效准则Tsai-W u failure criterion 达格代尔模型Dugdale model断裂力学fracture mechanics概率断裂力学probabilistic fractureMechanics格里菲思理论Griffith theory线弹性断裂力学linear elastic fracturemechanics, LEFM弹塑性断裂力学elastic-plastic fracturemecha-nics, EPFM 断裂Fracture 脆性断裂brittle fracture解理断裂cleavage fracture蠕变断裂creep fracture延性断裂ductile fracture晶间断裂inter-granular fracture 准解理断裂quasi-cleavage fracture 穿晶断裂trans-granular fracture 裂纹Crack裂缝Flaw缺陷Defect割缝Slit微裂纹Microcrack折裂Kink椭圆裂纹elliptical crack深埋裂纹embedded crack[钱]币状裂纹penny-shape crack预制裂纹Precrack短裂纹short crack表面裂纹surface crack裂纹钝化crack blunting裂纹分叉crack branching裂纹闭合crack closure裂纹前缘crack front裂纹嘴crack mouth裂纹张开角crack opening angle,COA 裂纹张开位移crack opening displacement,COD裂纹阻力crack resistance裂纹面crack surface裂纹尖端crack tip裂尖张角crack tip opening angle,CTOA裂尖张开位移crack tip openingdisplacement, CTOD裂尖奇异场crack tip singularityField裂纹扩展速率crack growth rate稳定裂纹扩展stable crack growth定常裂纹扩展steady crack growth亚临界裂纹扩展subcritical crack growth 裂纹[扩展]减速crack retardation 止裂crack arrest 止裂韧度arrest toughness断裂类型fracture mode滑开型sliding mode张开型opening mode撕开型tearing mode复合型mixed mode撕裂Tearing 撕裂模量tearing modulus断裂准则fracture criterionJ积分J-integralJ阻力曲线J-resistance curve断裂韧度fracture toughness应力强度因子stress intensity factor HRR场Hutchinson-Rice-RosengrenField 守恒积分conservation integral 有效应力张量effective stress tensor 应变能密度strain energy density 能量释放率energy release rate内聚区cohesive zone塑性区plastic zone张拉区stretched zone热影响区heat affected zone, HAZ 延脆转变温度brittle-ductile transitiontempe- rature 剪切带shear band剪切唇shear lip无损检测non-destructive inspection 双边缺口试件double edge notchedspecimen, DEN specimen 单边缺口试件single edge notchedspecimen, SEN specimen 三点弯曲试件three point bendingspecimen, TPB specimen 中心裂纹拉伸试件center cracked tensionspecimen, CCT specimen 中心裂纹板试件center cracked panelspecimen, CCP specimen 紧凑拉伸试件compact tension specimen,CT specimen 大范围屈服large scale yielding 小范围攻屈服small scale yielding 韦布尔分布Weibull distribution 帕里斯公式paris formula空穴化Cavitation应力腐蚀stress corrosion概率风险判定probabilistic riskassessment, PRA 损伤力学damage mechanics 损伤Damage连续介质损伤力学continuum damage mechanics 细观损伤力学microscopic damage mechanics 累积损伤accumulated damage脆性损伤brittle damage延性损伤ductile damage宏观损伤macroscopic damage细观损伤microscopic damage微观损伤microscopic damage损伤准则damage criterion损伤演化方程damage evolution equation 损伤软化damage softening损伤强化damage strengthening损伤张量damage tensor损伤阈值damage threshold损伤变量damage variable损伤矢量damage vector损伤区damage zone疲劳Fatigue 低周疲劳low cycle fatigue应力疲劳stress fatigue随机疲劳random fatigue蠕变疲劳creep fatigue腐蚀疲劳corrosion fatigue疲劳损伤fatigue damage疲劳失效fatigue failure疲劳断裂fatigue fracture 疲劳裂纹fatigue crack疲劳寿命fatigue life疲劳破坏fatigue rupture疲劳强度fatigue strength 疲劳辉纹fatigue striations 疲劳阈值fatigue threshold 交变载荷alternating load 交变应力alternating stress 应力幅值stress amplitude 应变疲劳strain fatigue应力循环stress cycle应力比stress ratio安全寿命safe life过载效应overloading effect 循环硬化cyclic hardening 循环软化cyclic softening 环境效应environmental effect 裂纹片crack gage裂纹扩展crack growth, crackPropagation裂纹萌生crack initiation 循环比cycle ratio实验应力分析experimental stressAnalysis工作[应变]片active[strain] gage基底材料backing material应力计stress gage零[点]飘移zero shift, zero drift 应变测量strain measurement应变计strain gage应变指示器strain indicator应变花strain rosette应变灵敏度strain sensitivity 机械式应变仪mechanical strain gage 直角应变花rectangular rosette引伸仪Extensometer应变遥测telemetering of strain 横向灵敏系数transverse gage factor 横向灵敏度transverse sensitivity 焊接式应变计weldable strain gage 平衡电桥balanced bridge粘贴式应变计bonded strain gage粘贴箔式应变计bonded foiled gage粘贴丝式应变计bonded wire gage 桥路平衡bridge balancing电容应变计capacitance strain gage 补偿片compensation technique 补偿技术compensation technique 基准电桥reference bridge电阻应变计resistance strain gage 温度自补偿应变计self-temperaturecompensating gage半导体应变计semiconductor strainGage 集流器slip ring应变放大镜strain amplifier疲劳寿命计fatigue life gage电感应变计inductance [strain] gage 光[测]力学Photomechanics光弹性Photoelasticity光塑性Photoplasticity杨氏条纹Young fringe双折射效应birefrigent effect等位移线contour of equalDisplacement 暗条纹dark fringe条纹倍增fringe multiplication 干涉条纹interference fringe 等差线Isochromatic等倾线Isoclinic等和线isopachic应力光学定律stress- optic law主应力迹线Isostatic亮条纹light fringe光程差optical path difference 热光弹性photo-thermo -elasticity 光弹性贴片法photoelastic coatingMethod光弹性夹片法photoelastic sandwichMethod动态光弹性dynamic photo-elasticity 空间滤波spatial filtering空间频率spatial frequency起偏镜Polarizer反射式光弹性仪reflection polariscope残余双折射效应residual birefringentEffect 应变条纹值strain fringe value应变光学灵敏度strain-optic sensitivity 应力冻结效应stress freezing effect 应力条纹值stress fringe value应力光图stress-optic pattern暂时双折射效应temporary birefringentEffect 脉冲全息法pulsed holography透射式光弹性仪transmission polariscope 实时全息干涉法real-time holographicinterfero - metry 网格法grid method全息光弹性法holo-photoelasticity 全息图Hologram全息照相Holograph全息干涉法holographic interferometry 全息云纹法holographic moire technique 全息术Holography全场分析法whole-field analysis散斑干涉法speckle interferometry 散斑Speckle错位散斑干涉法speckle-shearinginterferometry, shearography 散斑图Specklegram白光散斑法white-light speckle method 云纹干涉法moire interferometry [叠栅]云纹moire fringe[叠栅]云纹法moire method 云纹图moire pattern离面云纹法off-plane moire method参考栅reference grating试件栅specimen grating分析栅analyzer grating面内云纹法in-plane moire method 脆性涂层法brittle-coating method条带法strip coating method坐标变换transformation ofCoordinates计算结构力学computational structuralmecha-nics 加权残量法weighted residual method 有限差分法finite difference method 有限[单]元法finite element method 配点法point collocation里茨法Ritz method广义变分原理generalized variationalPrinciple 最小二乘法least square method胡[海昌]一鹫津原理Hu-Washizu principle赫林格-赖斯纳原理Hellinger-ReissnerPrinciple 修正变分原理modified variationalPrinciple 约束变分原理constrained variationalPrinciple 混合法mixed method杂交法hybrid method边界解法boundary solution method 有限条法finite strip method半解析法semi-analytical method协调元conforming element非协调元non-conforming element混合元mixed element杂交元hybrid element边界元boundary element 强迫边界条件forced boundary condition 自然边界条件natural boundary condition 离散化Discretization离散系统discrete system连续问题continuous problem广义位移generalized displacement 广义载荷generalized load广义应变generalized strain广义应力generalized stress界面变量interface variable 节点node, nodal point [单]元Element角节点corner node边节点mid-side node内节点internal node无节点变量nodeless variable 杆元bar element桁架杆元truss element 梁元beam element二维元two-dimensional element 一维元one-dimensional element 三维元three-dimensional element 轴对称元axisymmetric element 板元plate element壳元shell element厚板元thick plate element三角形元triangular element四边形元quadrilateral element 四面体元tetrahedral element曲线元curved element二次元quadratic element线性元linear element三次元cubic element四次元quartic element等参[数]元isoparametric element超参数元super-parametric element 亚参数元sub-parametric element节点数可变元variable-number-node element 拉格朗日元Lagrange element拉格朗日族Lagrange family巧凑边点元serendipity element巧凑边点族serendipity family无限元infinite element单元分析element analysis单元特性element characteristics 刚度矩阵stiffness matrix几何矩阵geometric matrix等效节点力equivalent nodal force 节点位移nodal displacement节点载荷nodal load位移矢量displacement vector载荷矢量load vector质量矩阵mass matrix集总质量矩阵lumped mass matrix相容质量矩阵consistent mass matrix 阻尼矩阵damping matrix瑞利阻尼Rayleigh damping刚度矩阵的组集assembly of stiffnessMatrices载荷矢量的组集consistent mass matrix质量矩阵的组集assembly of mass matrices 单元的组集assembly of elements局部坐标系local coordinate system局部坐标local coordinate面积坐标area coordinates体积坐标volume coordinates曲线坐标curvilinear coordinates静凝聚static condensation合同变换contragradient transformation 形状函数shape function试探函数trial function检验函数test function权函数weight function样条函数spline function代用函数substitute function降阶积分reduced integration零能模式zero-energy modeP收敛p-convergenceH收敛h-convergence掺混插值blended interpolation等参数映射isoparametric mapping双线性插值bilinear interpolation小块检验patch test非协调模式incompatible mode节点号node number单元号element number带宽band width带状矩阵banded matrix变带状矩阵profile matrix带宽最小化minimization of band width 波前法frontal method子空间迭代法subspace iteration method 行列式搜索法determinant search method 逐步法step-by-step method纽马克法Newmark威尔逊法Wilson拟牛顿法quasi-Newton method牛顿-拉弗森法Newton-Raphson method 增量法incremental method初应变initial strain初应力initial stress切线刚度矩阵tangent stiffness matrix 割线刚度矩阵secant stiffness matrix 模态叠加法mode superposition method 平衡迭代equilibrium iteration子结构Substructure子结构法substructure technique 超单元super-element网格生成mesh generation结构分析程序structural analysis program 前处理pre-processing后处理post-processing网格细化mesh refinement应力光顺stress smoothing组合结构composite structure。
ECS J. Solid State Sci. Technol.-2013-Vas-Umnuay-P120-9

P120ECS Journal of Solid State Science and Technology ,2(4)P120-P129(2013)2162-8769/2013/2(4)/P120/10/$31.00©The ElectrochemicalSocietyGrowth Kinetics of Copper Sulfide Thin Films by Chemical Bath DepositionParavee Vas-Umnuay and Chih-hung Chang ∗,zSchool of Chemical,Biological,and Environmental Engineering,Oregon State University,Corvallis,Oregon 97331,USACopper sulfides (Cu x S)are compound semiconductor materials that exhibit considerable variations of optical and electrical properties.Copper sulfide thin films can be used in many applications,such as solar control coatings,solar cells,photothermal conversion of solar energy,electroconductive coatings,and microwave shielding coatings.In this paper,chemical bath deposition growth of copper sulfide thin films were monitored for the first time using an in-situ quartz crystal microbalance as a function of time,temperature,concentrations of reactants,and pH.The reaction activation energy was determined based on initial growth rates.The high activation energy,68[kJ/mol],indicates that the rate limiting step of the deposition is the chemical reaction rather than mass transport.The structure,morphology,composition and optical absorption of the films were studied by scanning electron microscopy,transmission electron microscopy,energy dispersive X-ray spectroscopy and UV-Vis absorption spectroscopy respectively.These properties were found to depend strongly on the deposition conditions.©2013The Electrochemical Society.[DOI:10.1149/2.008304jss ]All rights reserved.Manuscript submitted November 27,2012;revised manuscript received January 8,2013.Published January 28,2013.Metal chalcogenide thin films,like metal sulfide,metal selenides,and metal tellurides,possess useful electrical and optical properties and can be found in many technical applications.Copper sulfide (Cu x S)thin films are one of the potentially useful metal chalco-genides with signification variation in properties depending on the stoichiometry,1≤x ≤2.At room temperature,five stable phases of Cu x S are known to exist in the bulk form:CuS (covellite),Cu 1.75S (anilite),Cu 1.8S (digenite),Cu 1.96S (djurleite),Cu 2S (chalcocite).1The different phases of Cu x S exhibit considerable variations of optical and electrical properties,therefore they can be used in different potential applications,such as solar control coatings,solar cells,photothermal conversion of solar energy,electroconductive coatings,microwave shielding coatings,etc.2Copper sulfide thin films could be prepared by a variety of meth-ods,including solution-based techniques (for example,successive ionic layer adsorption and reaction (SILAR),photochemical depo-sition,electrodeposition,chemical bath deposition (CBD),etc.)and gas phase techniques (for example,chemical vapor deposition (CVD),thermal co-evaporation,sputtering,etc.).Solution-based techniques require simpler and much lower capital cost equipment.Therefore,solution-based deposition methods are being considered as low-cost alternatives to gas phase techniques.3–6Among various solution-based techniques,CBD is the most widely used technique for the deposition of Cu x S thin films.4,7–16In a typical batch CBD process,Cu x S thin films are deposited by simply immers-ing the substrates in a dilute solution containing copper and sulfur reactive species.The reaction is thermally activated.There are a few reports regarding the growth kinetics of CBD Cu x S thin films.Munce et al.investigated chemical bath deposition of copper sulfide thin films in details using a plethora of in-situ and ex-situ characterization tools including Surface Enhanced Raman Scattering (SERS),Scan-ning Electron Microscopy (SEM),Transmission Electron Microscopy (TEM),Neutron reflectometry,and small angle X-ray scattering to in-vestigate the early stages of film growth.11,17,18The deposition of CBD Cu x S was found to occur by a mechanism where the particles form in the solution and then adhere to the substrate according to the lack of a definable SERS spectrum prior to 1min deposition.While during the incubation period,the spectra obtained from the nucleation products exhibited a similar composition to the final product formed on the substrate.Another Cu x S film growth study was reported by Xin et al.2They compared the Cu x S film growth by the conventional CBD with the microwave assisted CBD by measuring the film thickness at cer-tain deposition times using the profilometer as an ex-situ technique.They found that the high frequency electromagnetic radiation of mi-crowave heating accelerated the growth rate of copper sulfide thin∗Electrochemical Society Active Member.zE-mail:changch@films,resulting in thicker films at the same reaction time compared with the conventional CBD method.Moreover,Lu et al.investigated the growth study of Cu x S thin films by depositing CuS thin films by CBD on functionalized -NH 2-terminated self-assembled monolayers (SAMs)surface which helps to control crystal heterogeneous nucle-ation and growth.4The reaction mechanism of ion-by-ion growth and cluster-by-cluster deposition were proposed for the observed depo-sition phenomena.There are a number of papers that report copper sulfide thin film growth by CBD,with different bath compositions and conditions (i.e.acidic or alkaline).9,12,13,19–21However,no quantitative kinetic study of CBD Cu x S thin film growth has been reported in these studies.In-situ measurements are valuable and effective means to gain a better and more quantitative understanding of CBD Cu x S thin film growth mechanism and kinetics.In this paper,we investigated CBD Cu x S thin film growth mecha-nism using in-situ quartz crystal microbalance (QCM)by considering the initial values of the growth rate as a function of the main reac-tion parameters (temperature,concentrations of reactants,and pH).The resulting thin films were characterized by scanning electron mi-croscopy,transmission electron microscopy,energy dispersive X-ray spectroscopy,atomic force microscopy,and UV-Vis absorption.These characterizations together determine the composition,structural,and optical properties of the resulting thin films.ExperimentalSubstrate preparation.—Microscope glass slides (1×3inch from Fisher Scientific)were cleaned with a commercial detergent solution by scrubbing followed by rinsing with acetone,methanol,and deion-ized water.Clean substrates were dried in flowing nitrogen.Deposition of Cu x S thin films.—Various deposition conditions were performed in this study.A typical growth procedure is describe here as an example.8mL of 1M CuSO 4.5H 2O,8mL of 1M sodium acetate (NaAC)solution,8mL of triethanolamine (TEA)solution were mixed in a 100mL beaker.This was followed by the addition of 4.44mL of 28%ammonia solution and 4.44mL of 1M thiourea solution.The resulting solution was made up to 80mL with deionized water,resulting in the final concentration of each reactant as follows:[CuSO 4]=0.1M,[thiourea]=0.056M,[NaAc]=0.1M,[TEA]=0.755M,and [NH 3]=0.821M.Stirring and heating the mixture were applied in a water bath by a hot plate.After a few seconds the glass slide was immersed vertically in the solution bath.At the end of the deposition the coated substrates were taken out,rinsed with deionized water,and dried in nitrogen.ECS Journal of Solid State Science and Technology,2(4)P120-P129(2013)P121 In-situ growth measurement.—Thefilm thickness and growth ratebased on different bath conditions was measured using a quartz crystalmicrobalance(QCM,Maxtek incorporated Model at a frequency of5MHz).The setup consisted of a QCM probe with a quartz crystalinserted inside and immersed in the solution bath.The signal from thequartz crystal probe is sent to the quartz crystal monitor and computerfor data acquisition.Characterization of Cu x S thinfilms.—The Cu x S thin-film surfacemorphology,composition,and structure were analyzed by scanningelectron microscopy(SEM)coupled with the X-ray analysis(EDS)(Quanta600FEG SEM).The formed nanoparticles in the solutionwere analyzed using transmission electron microscopy(TEM,PhilipsCM12STEM operated at120kV).The crystal structure of CBDCu x Sfilms were characterized using high resolution TEM(FEI Titan80–200STEM).The surface morphology was analyzed using atomicforce microscope(AFM Veeco Innova).The optical transmission and optical bandgap of CBD Cu x S thinfilms were determined using UV-VIS absorption measurement(JASCO V670spectrophotometer).Results and DiscussionInitial growth rate dependent.—Influence of temperature.—The composition of the baseline solution used in this study is[CuSO4] =1M,[thiourea]=1M,[TEA]=7.55M,and[NH3]=14.8M.Thegrowth curves obtained as a function of bath temperature are shown in Fig.1a.The solution temperature is varied with an increment of 10◦C,from30to60◦C.The growth curve shows an initial induction phase where nofilm growth can be observed by QCM.Following the induction period,a subsequent linear growth phase starts with the increasingfilm thickness proportionally with deposition time.Finally,film thickness reaches a plateau where the growth rate drops to nearly zero.The growth curves clearly show strong temperature dependence. The induction period at30◦C lasts for almost10minutes but decreases to1–2minutes at60◦C.Fig.1b shows a growth curve with three growth regimes(i.e.induction,growth,and terminal regime)for CBD Cu x S film growth at60◦C.The initialfilm growth rates were estimated by taking the slope of the linear growth curves.The reacting species concentration within the initial linear growth region was assumed to be constant.Since the process is strongly temperature-activated, its variation is attributed only to the rate constant dependence on temperature,as expressed by Arrhenius equationr(T)=A exp(−E a/RT)[1] where r(T)is the growth rate as a function of temperature,A is the pre-exponential factor which includes the frequency factor and a con-stant related to the initial reagent concentration,E a is the activation energy,and R is the molar gas constant.Fig.1c represents the Nepe-rian logarithm of the initial growth rate as a function of1000/T(K), showing the linear dependence of temperature.Each data point is the Neperian logarithm of growth rate which is calculated as the slopes at initial growth regions of different reaction temperatures,as shown in the inset of Fig.1c obtained from Fig.1a.Table I summarizes the initial growth rates at different temperatures.The activation energy can be determined from the slope of the linearfit of the logarithm of the initial growth rate as a function of1000/T.The mean value of the activation energy in this case is about68kJ/mol over the tempera-ture range considered.This value,which is considered high(50–100 [kJ/mol]),indicates that the rate determining step in thefilm deposi-tion mechanism is a reaction limiting step rather than a mass transport step(e.g.diffusion)that normally has a lower activation energy value (a few tens of kJ/mol).21Influence of copper concentration.—Fig.2a shows the growth rate variation with CuSO4concentration,which was varied from 0.5–1.25M while the other parameters are kept constant([thiourea] =1M,[TEA]=7.55M,[NH3]=14.8M at40◦C).An increase(a)(b)0.511.522.533.54020406080 Thickness(kAng)Time (min)30°C40°C50°C60°C0.511.522.533.544.55020406080 Thickness(kAng)Time (min)60°CI IIIIII II(c)y = -8.2045x + 28.495R² = 0.96811.522.533.544.52.9533.05 3.1 3.15 3.2 3.25 3.3 3.35ln(Rate(µm/hr))1000/T (K-1)Figure1.(a)Quartz crystal microbalance growth curves of Cu x Sfilms with different temperatures,(b)Growth curve of CBD Cu x Sfilm at60◦C with three deposition regimes:I=induction regime;II=growth regime;III=terminal regime,and(c)Neperian logarithm of the initial growth rate against1000/T (K−1)with[CuSO4]=1M,[thiourea]=1M,[TEA]=7.55M,and[NH3] =14.8M with the inset showing the initial slopes(growth rates)of Fig.1(a).of growth rate with CuSO4concentration is observed.The induction time also becomes shorter at higher CuSO4concentration.In order to determine the apparent reaction orders,the growth rate is assumed to be dependent on the variation of initial reagentP122ECS Journal of Solid State Science and Technology ,2(4)P120-P129(2013)Table I.Summary of the initial growth rates at different temperatures,[CuSO 4],[Thiourea],[TEA],pH values,and [NH 3].Temperature,◦CGrowth rate (slope),kÅ/min[CuSO 4],mol/lGrowth rate (slope),kÅ/minCondition:[CuSO 4]=1M,[thiourea]=1M,Condition:[thiourea]=1M,[TEA]=7.55M,[TEA]=7.55M,and [NH 3]=14.8Mand [NH 3]=14.8M at 40◦C.300.00780.500.010400.01500.750.013500.0320 1.000.015600.0920 1.250.016[thiourea],mol/l Growth rate (slope),kÅ/min[TEA],mol/l Growth rate (slope),kÅ/minCondition:[CuSO 4]=1M,[TEA]=7.55M,Condition:[CuSO 4]=1M,[thiourea]=1M,and [NH 3]=14.8M at 40◦Cand [NH 3]=14.8M at 40◦C0.500.009 1.000.02870.750.013 2.500.0201.000.015 5.000.0161.250.0167.550.015pH Growth rate (slope),kÅ/min[NH 3],mol/l Growth rate (slope),kÅ/minCondition:[CuSO 4]=1M,[thiourea]=1M,Condition:[CuSO 4]=1M,[thiourea]=1M,[TEA]=7.55M,and [NH 3]=14.8M at 40◦Cand [TEA]=7.55M at 40◦C9.920.0065 5.00.00859.990.009210.00.011010.050.010613.00.013010.100.015014.80.0150concentration during the initial linear growth region.The initial growth rate is expressed as in Eq.2.21r =K C ni [2]where r is the growth rate which can be estimated from the slopes of linear growth curves as shown in the inset of Fig.2b .K is a constant(a)(b)00.20.40.60.811.21.41.61.8020406080100120T h i c k n e s s (k A n g )Time (min)[CuSO 4] 0.5 M [CuSO 4] 0.75M [CuSO 4] 1.0 M [CuSO 4] 1.25 My = 0.5217x -1.0554R² = 0.9794-1.25-1.2-1.15-1.1-1.05-1-0.4-0.3-0.2-0.100.10.2l o g (r a t e ) (µm /h r )log[CuSO 4] (mol/l)Figure 2.(a)Influence of CuSO 4concentration (0.5,0.75,1,and 1.25M)on the growth curves of Cu x S films with samples prepared with [thiourea]=1M,[TEA]=7.55M,and [NH 3]=14.8M at 40◦C.(b)The corresponding logarithmic graph for reaction order calculations of the initial growth rate dependence on CuSO 4concentration with the inset showing the initial slopes (growth rates)of Fig.2(a).for a fixed set of conditions,C i is the initial reagent concentration that is being varied,and n is the reaction order of this reagent.Therefore,n can be estimated for each reagent as the slope of the linear fit of the log r vs.log[C i ].The summary of the initial growth rates at different concentration of CuSO 4is shown in Table I and Fig.2b shows the linear plot of film growth rate as function of the concentration of CuSO 4.The mean apparent reaction order of the CuSO 4concentration was estimated to be about 0.52±0.2.Influence of thiourea concentration.—Fig.3a shows the influence on the growth curves of the thiourea concentration from 0.5–1.25M while the other parameters are kept constant ([CuSO 4]=1M,[TEA]=7.55M,[NH 3]=14.8M at 40◦C).An increase of the initial growth rate with the concentrations is observed as expected,as shown in the inset of Fig.3b .The summary of the initial growth rates at different concentration of thiourea is shown in Table I .A reaction order of 0.63±0.1is obtained from the slope of the linear fit of the log r vs.log[thiourea]shown in Fig.3b .Copper ions (Cu 2+),introduced in solution as copper salts,can form different complex species with complexing agents such as TEA and ammonia.Appropriate complexing agents are present to produce stable complex of Cu 2+ions in the solution in which Cu 2+ions are slowly released on dissociation,resulting in a controllable reaction rate.For a metal M and complexing agent A,the existence of free metal ions in the solution can be expressed by the equilibrium reactionM(A)2+↔M 2++A[3]Influence of TEA concentration.—Fig.4a shows the growth curves of Cu x S thin film as a function of TEA concentration from 1–7.55M while the other parameters are kept constant ([CuSO 4]=1M,[thiourea]=1M,[NH 3]=14.8M at 40◦C).A decrease of the growth rate is observed with increasing TEA concentration,as shown in the inset of Fig.4b ,in which it retards the rate of formation of Cu x S.The immediate homogeneous precipitation appears sooner in the solution as the concentration decreases,also yields less terminal thicknesses of the films.The summary of the initial growth rates at different concentration of TEA is shown in Table I .The effect of TEA concentration shows opposite effect to those observed for the CuSO 4and thiourea,as the reaction rate shown in Fig.4b decreases with in-creasing TEA concentration,with an apparent order of −0.33±0.2.In this case,an increase in TEA concentration results in a decrease inECS Journal of Solid State Science and Technology ,2(4)P120-P129(2013)P123(a)(b)00.20.40.60.811.21.41.61.8020406080100120140160T h i c k n e s s (k A n g )Time (min)[TU] 0.5 M [TU] 0.75 M [TU] 1.0 M [TU] 1.25 My = 0.6347x -1.0575R² = 0.9518-1.28-1.23-1.18-1.13-1.08-1.03-0.35-0.25-0.15-0.050.05l o g (r a t e ) (µm /h r )log[TU] (mol/l)Figure 3.(a)Influence of thiourea concentration (0.5,0.75,1,and 1.25M)on the growth curves of Cu x S films with samples prepared with [CuSO 4]=1M,[TEA]=7.55M,and [NH 3]=14.8M at 40◦C.(b)The corresponding logarithmic graph for reaction order calculations of the initial growth rate dependence on thiourea concentration with the inset showing the initial slopes (growth rates)of Fig.3(a).growth rate,corresponding to Eq.3,which denotes that the Cu 2+free concentration decreases when TEA concentration is increased.Influence of pH.—In this study,the initial pH value of the solution was controlled by the addition of 1M of NaOH while keeping other parameters constant ([CuSO 4]=1M,[thiourea]=1M,[TEA]=7.55M,[NH 3]=14.8M at 40◦C).As observed in Fig.5a ,an increase of pH value leads to a higher film growth rate.Higher concentration of OH −ions in the solution will push the re-action of thiourea hydrolysis forward,resulting in a high generation of sulfide ion source,as shown in Eq.4–5.Table I summarizes the initial growth rates at different pH values,which were obtained from the slope at initial growth regions as shown in the inset of Fig.5b .A mean fractional apparent order of about 1.91±0.1is obtained as shown in Fig.5b .(NH 2)2CS +OH −↔CH 2N 2+H 2O +HS −[4]HS −↔S 2−+H +[5]Influence of NH 3concentration.—Fig.6a shows the Cu x S growthcurves as a function of ammonia concentration from 5–14.8M while keeping other parameters constant at [CuSO 4]=1M,[thiourea]=1M,[TEA]=7.55M,at 40◦C.The effect of ammonia concen-tration was expected to behave similarly to TEA as they both act as(a)(b)0.20.40.60.811.21.41.60102030405060708090100T h i c k n e s s (k A n g )Time (min)[TEA] 1 M[TEA] 2.5M [TEA] 5.0 M[TEA] 7.55 My = -0.3283x -0.775R² = 0.9828-1.05-1-0.95-0.9-0.85-0.8-0.75-0.700.20.40.60.81l o g (r a t e ) (µm /h r )log[TEA] (mol/l)Figure 4.(a)Influence of TEA concentration (1,2.5,5,and 7.55M)on the growth curves of Cu x S films with samples prepared with [CuSO 4]=1M,[thiourea]=1M,and [NH 3]=14.8M at 40◦C.(b)The correspond-ing logarithmic graph for reaction order calculations of the initial growth rate dependence on TEA concentration with the inset showing the initial slopes (growth rates)of Fig.4(a).complexing agents.The inset of Fig.6b shows the slopes of the growth curves in the linear growth region.The summary of the initial growth rates at different concentration of ammonia is shown in Table I .It has been reported by Mondal et al.that complexing the Cu 2+ions with TEA first,and then adding ammonia yields better uniformity of films with a maximum terminal thickness.22,23An apparent reaction order of 0.49±0.2was calculated according to Fig.6b .Normally,the addition of complexing agent decreases the reaction rate.The role of ammonia is not only to control the complexation of copper ions but also to provide OH −ions according to the equilibrium reaction expressed in Eq.6.Thus addition of ammonia tends to increase the growth rate.NH +4+OH −↔NH 3+H 2O[6]Since the ammonia concentration variation affects the pH of thesolution,the reaction order cannot be determined directly with respect to ammonia concentration.The reaction order obtained in Fig.6b is taken as an apparent reaction order affected by pH variation.In order to derive a real reaction order of ammonia,the ammonia hydrolysis equilibrium is taken into account,21we can writeK b =10−4.8=[NH +4][OH −]/[NH 3][7]For nonbuffered solutions [NH 4+]=[OH −],hence[OH −]=(10−4.8[NH 3])1/2[8]P124ECS Journal of Solid State Science and Technology ,2(4)P120-P129(2013)(a)00.20.40.60.811.21.41.6102030405060708090100T h i c k n e s s (k A n g )Time (min)pH 9.92pH 9.99pH 10.05pH 10.10(b)y = 1.9078x -20.334R² = 0.9711-1.42-1.37-1.32-1.27-1.22-1.17-1.12-1.07-1.029.99.951010.0510.110.15l o g (r a t e ) (µm /h r )pHFigure 5.(a)Influence of pH (9.92,9.99,10.05,and 10.10)on the growth curves of Cu x S films with samples prepared with [CuSO 4]=1M,[thiourea]=1M,[TEA]=7.55M,and [NH 3]=14.8M at 40◦C.(b)The corresponding logarithmic graph for reaction order calculations of the initial growth rate dependence on pH with the inset showing the initial slopes (growth rates)of Fig.5(a).Using Eq.2for ammonia,we can writer =K [NH 3]na[9]where na is an apparent order and Eq.9can be rewritten asr =K [NH 3]nr [OH −]1.91[10]where nr is a real reaction order of ammonia.Eq.10can be rewritten using Eq.8r =K [NH 3](nr +0.96)[11]so nr can be calculated asnr =na −0.96[12]which gives place to a reaction order of −0.47for ammonia.The negative apparent reaction order indicates that the role of ammonia complexing with Cu 2+ions,which lowers the reaction rate when the ammonia concentration increases,dominates the effects of the variation of pH in the solution.The initial growth rate is given approximately by the following empirical equationrate of reaction (μm /hr)=K[CuSO 4]0.52[TU]0.63[OH −]1.91[TEA][NH 3][13]K =2.94×106[μm7.78/mol2.26.hr]at 40◦CMorphology dependence of Cu x S thin films on deposition conditions.—The results of visual examination and Scotch tape test(a)00.20.40.60.811.21.41.61.8020406080100120140160T h i c k n e s s (k A n g )Time (min)[NH 3] 5 M [NH 3] 10M [NH 3] 13 M [NH 3] 14.8 M(b)y = 0.4928x -1.6474R² = 0.9557-1.3-1.25-1.2-1.15-1.1-1.05-10.60.70.80.91 1.1 1.2l o g (r a t e ) (µm /h r )log[NH 3] (mol/l)Figure 6.(a)Influence of [NH 3]concentration (5,10,13,and 14.8M)on the growth curves of Cu x S films with samples prepared with [CuSO 4]=1M,[thiourea]=1M,and [TEA]=7.55M at 40◦C.(b)The correspond-ing logarithmic graph for reaction order calculations of the initial growth rate dependence on NH 3concentration with the inset showing the initial slopes (growth rates)of Fig.6(a).of the obtained films indicate that glass substrate provides suitable nucleation sites for the growth of Cu x S thin films with good adhesion of the film to the substrate surface.The microstructure and crystalline structure of the Cu x S films prepared by CBD were studied by SEM and TEM.SEM micrographs of samples prepared from the composi-tion of [CuSO 4]=1M,[thiourea]=2.02M,[TEA]=7.55M,and [NH 3]=14.8M at 40◦C with different deposition time (30,40,and 60min)are shown in Fig.7.These micrographs display densely packed films with granular morphologies.The grain sizes increase with an increase of deposition time,ranging from about 70–200nm.More large particles appear on the surface at longer deposition time.These large particles might come from the sticking of particles formed in the solution.The surface roughness of Cu x S thin films was examined by atomic force microscopy (AFM).The inset images in Fig.7a -7c show the evolution of root mean square roughness (RMS),RMS is defined as the standard deviation of the surface height profile from the average height,of 32.0,32.8,and 36.7nm of the films deposited for (a)30min,(b)40min,and (c)60min,respectively.The results from the AFM are consistent with the results from the SEM accordingly,showing the formation of larger size grains at longer deposition time.A cross-sectional SEM image of the resulting Cu x S thin film de-posited on glass substrate for 60min is shown in Fig.8.The measured thickness is about 80nm which corresponds to the QCM growth curve obtained earlier for the bath containing [CuSO 4]=1M,[SC(NH 2)2]=2.02M,[TEA]=7.55M,and [NH 3]=14.8M at 40◦C.The cross-sectional SEM also shows larger particles on top of the film.TheECS Journal of Solid State Science and Technology,2(4)P120-P129(2013)P125Figure7.SEM micrographs of Cu x Sfilms prepared at(a)30min,(b)40min, and(c)60min with[CuSO4]=1M,[SC(NH2)2]=2.02M,[TEA]=7.55 M,and[NH3]=14.8M at40◦C.The insets show AFM images of Cu x S thin films deposited for30min,40min,and60min accordingly.observedfilm thickness is low because part of the reacting species is lost to the competing formation of Cu x S particles in the solution.We deposited Cu x S heterogeneously on the thin lacey carbon sur-face of TEM copper grid.Thefilm was prepared by dipping the grid in a batch solution at40◦C for15minutes.The TEM image of Cu x S given in Fig.9a shows a large collection of rice-shaped nanoparticles. Fig.9b shows the corresponding a high resolution image of the Cu xS Figure8.Cross-sectional SEM image of Cu x S deposited for60min,with [CuSO4]=1M,[SC(NH2)2]=2.02M,[TEA]=7.55M,and[NH3]=14.8 M at40◦C.Figure9.High resolution TEM images of Cu x S thinfilms from a heteroge-neous reaction prepared by dipping the copper grid in hot solution for15min at40◦C.(a)low resolution image of Cu x Sfilm,(b)high resolution image of the lattice fringes,and(c)FFT pattern.P126ECS Journal of Solid State Science and Technology ,2(4)P120-P129(2013)Figure 10.TEM micrographs of Cu x S nanoparticles from CBD bath after (a)10min,(b)15min,(c)30min,and (d)40min of deposition time.nanocrystal.The image shows a number of nanocrystals at the order of a few nanometers on the grid with clearly observed lattice image.The corresponding fast Fourier transform (FFT)image is given in Fig.9c .The observed lattice-plane spacing-d values are in good agree-ment with the JCPDS card no.06-0464for the hexagonal phase of CuS with a =3.792Åand c =16.344Å.Cu x S particles formed homogeneously in the solution were also studied by TEM as a function of deposition time.TEM images of nanoparticles taken from the solution at the deposition time of 10,15,30,and 40min are given in Fig.10a -10d .It can be clearly observed from the micrograph shown in Fig.10a that the very small particles were formed in the solution at the beginning of the process,showingECS Journal of Solid State Science and Technology,2(4)P120-P129(2013)P127Figure11.SEM micrographs showing the influence of[CuSO4]/[SC(NH2)2] ratio on the surface morphology of the50min deposited Cu x Sfilms prepared at40◦C.(a)0.100:0.112,(b)0.100:0.056,and(c)0.100:0.028.a speckled appearance of spherical particles.At15min,these small particles(<10nm)start to aggregate with each other and form a network(Fig.10b).At this stage there is no obvious single particle forming and growing.At30min(Fig.10c),larger particles with a size of about30–50nm were observed.Particles continue to grow to60–80nm at40minutes of deposition time(Fig.10e).The large particles tend to aggregate together and form clusters which might12345678090100300800130018002300T(%)Wavelength (nm)(a)30 min(b)40 min(c)50 min(d)60 min(e)90 min(f)120 minFigure12.Optical transmission spectra of as-prepared Cu x Sfilms,deposited at40◦C with durations of deposition ranging from30–120min.(The inset image shows thefilm appearance at50min).correlate with the large particles observed in the SEM micrographs (Fig.7c-7d).Figure11a-11c shows SEM micrographs of Cu x Sfilms prepared under the conditions consisting offinal[TEA]=0.755M,[NH3] =0.821M at40◦C by varying thefinal[CuSO4]/[SC(NH2)2]ratio in the reaction bath(0.100:0.112,0.100:0.056,and0.100:0.028M, respectively)with a deposition time of50minutes.It is observed that for the ratios of0.100:0.112,where thiourea is in an excess of copper concentration,thefilm is continuous,compact with good coverage of the substrate,as shown in Fig.11a.Whereas thefilms obtained at ratio of0.100:0.056and0.100:0.028,when the concentration of thiourea is less than copper,thefilms do not fully cover the glass substrate surface,as shown in Fig.11b-11c.This result coincides with thefilm appearance in which thefilm is discontinuous across the substrate surface.According to these observations,we believe that Cu x Sfilm deposition requires the formation of meta-stable complex of copper as an anchoring site for the thiourea molecule adsorption. Therefore,when there is an excess of thiourea molecules compared to the copper sites,all of copper sites could be occupied,resulting in the uniform formation of Cu x Sfilm across the substrate surface. On the contrary,in a thiourea deficient condition not all the copper sites would be occupied,resulting in nonuniformly coveredfilms. This result clearly shows that the proper copper to thiourea ratio is important for obtaining continuousfilm coverage.Optical studies.—The optical transmittance and optical bandgap of the Cu x S thinfilms were measured using a UV-visible spectropho-tometer.The optical transmission spectra of the Cu x Sfilms,pre-pared from a solution with a[CuSO4]to[SC(NH2)2]molar ratio of0.100:0.056at40◦C at various deposition time ranging from30to 120minutes,are shown in Fig.12.The as-depositedfilms visually look uniform and transparent with a metallic appearance(see the inset in Fig.12).Thefilms show high transmittance in the visible region and the transmittance decrease for thefilms obtained at longer de-position times.This could be attributed to increasingfilm thickness and the change of stoichiometry of resulting Cu x S thinfilms.Optical transmission measurements were also performed forfilms obtained from chemical baths with various[CuSO4]/[SC(NH2)2]molar ratios at40◦C.The spectra fromfilms with a60minute deposition time and[CuSO4]/[SC(NH2)2]molar ratio of0.050:0.056,0.075:0.056, 0.100:0.056,0.100:0.042,0.100:0.028are given in Figure13a,13b, 13c,13d,and13e respectively.These spectra indicate that the Cu x S films have high transmittance after the absorption edge at about 670nm throughout the visible and near-infrared region.Lower trans-mittance is observed fromfilms deposited from baths with a higher concentration of copper and thiourea.。
Conversion_of_solar_heat_into_work_A_supplement_to_the_actual_thermodynamic_description

Conversion of solar heat into work:A supplement to the actual thermodynamic descriptionptevInstitute of Physical Electronics(IPE),University of Stuttgart,Pfaffenwaldring47,70569Stuttgart,Germany͑Received13July2005;accepted7November2005;published online23December2005͒The absorption of radiant energy in solar cells is divided into reversible and irreversible processes occurring in parallel.The paper proposes a method for calculating their relative contributions.We also show that photon reemission without work production plays a particular role in the conversion of solar heat into work and the attainment of higher solar cell efficiencies.The comparison of the well-known thermodynamic efficiency limitations of the solar energy conversion with and without entropy production makes it possible to formulate the notion“antenna states of the absorber particles.”The carriers of similar states are known as pigment molecules in the photosynthesis of plants.We show that it is impossible to attain very high efficiencies in the conversion of solar heat into work without the reversible antenna reemission of solar energy.©2005American Institute of Physics.͓DOI:10.1063/1.2149189͔I.INTRODUCTIONModern thermodynamic descriptions of the conversion of solar heat into work are made in two different ways.The first is to draw up a balance sheet of energy and entropy fluxes.1–5The second is to use the method of cycles in order to give a visual description of the solution of balance equations.3–7One looks for paths along which the energy exchange between radiation and matter is accompanied by the performance of a maximum work.The work is maximum when a quasi-static process takes place in a device.However, substance and radiation are never in equilibrium in solar cells and quasi-static conversion of the solar energy is not achieved.For this reason,the optimal configuration for various irreversible thermodynamic engines is determined using,for example,the method of endoreversible thermodynamics of solar energy conversion.8Endoreversible engines are irre-versible engines where all irreversibilities are restricted to the coupling of the engine to the external world.9It is as-sumed that the inner reversible part of an endoreversible en-gine is a Carnot cycle.This work proposed to look for a continuous series of equilibrium states outside the irreversible or endoreversible engine,isolate these states into separate processes,and use them to obtain a higher efficiency of the generally non-quasi-static solar energy conversion.We can consider those equi-librium processes as a base of the“exoreversible”additional device for the irreversible or endoreversible engine.The ther-modynamical maximum work principle defined in this way was not employed in earlier work on the conversion of radi-ant energy.1–11Physics of the solar energy conversion is based also on the theory of zones,which nowadays has been used most frequently in the calculation of the solar cell efficiency.12–17 Other authors use the theory of zones and the principles of thermodynamics͑chemical potential͒concurrently.18,19Inthis work we combine only the principles of thermodynamicsand quantum theory for the cycle processes.ING MODEL FOR CONVERTING RADIANT ENERGY INTO WORKIn this article,we use the well-known model of a solarenergy convertor shown in Fig.1.19The heat radiation ab-sorber͑1͒is black body with temperature T A and placed inthe center of the spherical cavity͑2͒with mirror walls andlens͑3͒,which makes use of optical methods in order tobring about a maximum concentration of radiation on theblack surface.The heat receiver͑4͒with temperature T0͑less than T A͒is in contact with the black body.The mirror͑5͒regulates the penetration of solar radiation into cavity͑2͒.Ifthe mirror is in the position shown in Fig.1,the cavity con-tains radiations with temperatures T A and T S.If the mirrorblocks the penetration of solar light,the cavity contains onlyradiation from black body͑1͒.Other types of radiation in thecavity are not considered here.In this model,radiant energyis converted at the limit temperatures T0=300K and T S=5800K.The black body has a temperature of T A=320K.III.ENERGY EXCHANGE BETWEEN RADIATIONAND MATTERThe solar radiation in cavity͑2͒with volume V has en-ergy U S=VT S4and entropy S s=4VT S3/3,whereis the Stefan-Boltzmann constant.The black body absorbs the ra-diation and emits radiation with energy U A=VT A4in cavity ͑2͒.If T A=320K,these energies stand in a ratio of U S/U A Ϸ106,while S S/S AϷ6ϫ103.As the volumes of radiations are equal,the amount of evolved heat⌬Q is proportional to the difference T A4−T S4and is equal to the area under the iso-chore st on the entropy diagram drawn on the plane formed by the temperature͑T͒and entropy͑S͒axes͑Fig.2͒.The ratio of the heat⌬Q to the solar energy U S entering the cavity isJOURNAL OF APPLIED PHYSICS98,124905͑2005͒0021-8979/2005/98͑12͒/124905/5/$22.50©2005American Institute of Physics98,124905-1U =͑U S −U A ͒/U S =1−͑T A /T S ͒4.͑1͒In our model,U is close to 1for ͑T A /T S ͒4ϳ10−5.A.Work produced by radiation and matterin Carnot cyclesThe absorbed radiant heat is converted into work by Car-not cycles involving matter or radiation.One such cycle is the rectangle abcd in Fig.2.Work is performed during this cycle with an efficiency of0=1−T 0/T A =0.0625͑2͒between the limit temperatures T 0=300K and T A =320K.Radiation performs work during the Carnot cycle with an efficiency greater than 0.Let us show the absorption ofradiation on an entropy diagram ͑Fig.3͒as an isothermaltransfer of radiation from the volume V2of the cavity ͑states ͒to the volume V 1of the black body ͑state p ͒.One can even reduce the radiation to state p *͑Fig.3͒.We will not discuss the properties of points p and p *here.Let us simply note that radiation reaches heat equilibrium with the black body ͑state e ͒from these points either through the adiabatic process p *e or through the isochoric process pe .Let us represent the emission of radiation as its transfer from the volume of the black body ͑state e ͒to the volume V 2of the cavity along the isotherm T A ͑state t ͒.As the radiation fills the cavity,it performs work equal to the difference be-tween the evolved and absorbed heat.The radiation performs considerable work if it reaches state t *in Fig.3.Our calcu-lations show that work is performed along the path sp *et *with an efficiency of C =1−T A /T S =0.945͑3͒when T A =320K.It is important to note that,when radiation returns to its initial state s along the adiabatic t *s ,it consti-tutes a Carnot cycle with the same efficiency C.FIG.2.Entropy diagram showing isochoric cooling of radiation ͑line st ͒in cavity ͑2͒.The amount of evolved radiant heat is proportional to the area sts t s s .The amount of heat converted into work is proportional to the area abcd .The work is performed by matter in a thermal engine during a Carnotcycle.FIG.3.Entropy diagram showing some thermodynamic cycles for conver-sion of solar heat into work in cavity ͑2͒with the participation of a black body.Isotherms represent the absorption and emission of radiant energy.Lines pe and p *e correspond to the cooling of radiation in the black body.Line st indicates the temperature and entropy of radiation in cavity ͑2͒.FIG.1.Model of solar energy conversion from Ref.19.Designations:͑1͒black body;͑2͒spherical cavity;͑3͒lens;͑4͒heat receiver;͑5͒movable mirror added by the author.B.Limits of the conversion of solar heat into work by reversible processes other than the Carnot cycleSolar energy is converted as a result of a combination of different processes.Their mechanisms are mostly unknown.For this reason,one tries to establish the temperature depen-dence of the limiting efficiency of a reversible combined process with the help of balance equations for energy and entropy flows.For solar radiation,it takes the form 4AS =1−4T A /3T S +T A 4/3T S 4.͑4͒For example,AS =0.926when T A =320K.The measure ofmagnitudeof L is C ,the efficiency of a Carnot cycle with the same temperature interval.The maximum difference C−AS is approximately 18%when T A =3500K.4The efficiency of a reversible process in which radiation and matter perform work is equal to 19L =1−͑T A /T S ͒4−4T 0͓1−͑T A /T S ͒3͔/3T S .͑5͒For example,L =0.931when T A =320K and T0=300K.The measure of magnitude of L is not indicated in Ref.19.C.Solar energy conversion as a combination of reversible and irreversible processesThe absorption of radiation precedes the conversion of solar heat into work.In our model,the black body absorbs solar radiation and generates another radiation with a smaller temperature.Heat is evolved in the process;it is either con-verted into work or irrevocably lost.For the sake of simplic-ity,let us assume that heat is lost with an efficiency of U from Eq.͑1͒.In Fig.4below,we give the efficiencies of the combinations of the different Carnot cycles in the presence of irreversible losses of radiant energy.In these cases,the conversion efficiencies become smaller:C U and 0U .When energy is converted by two simultaneous cycles,the efficiency becomes even smaller:0C U .In Fig.4,the tem-perature dependence of these values is represented by the lines KB ,CEB ,and CDB ,respectively.In the region below each line,the work obtained is reconverted into heat by irre-versible processes.D.The diagram of the solar cell efficiency as a reversible diagram The temperature dependence of L from Eq.͑5͒is shown by line LB in Fig.5.Let us also make use of the fact that this line is ͑by definition ͒a graphic illustration of the sequence of reversible transitions from one state of the sys-tem to another only.Then,the coordinates of the points on the lines representing the combinations of efficiencies of the reversible and irreversible processes ͑lines KB ,CDB ,and CEB in Figs.4and 5͒are proportional to their share of reversible transitions.For example,let point a in Fig.5denote the conversion of solar energy along the line CE with an efficiency of 30%.Let us draw an isotherm through point a ;the intersections of this line with the line LB and the y axis give us the values L =0.93and T A =430K.Then,by the lever principle,point a corresponds to ͑L −30͒100/L =68%irreversible pro-cesses and 32%reversible ones.The lines CEB and CDB in Figs.4and 5are not the only illustration of possible ways of converting solar energy.For example,it is known that the limiting efficiencies of solar elements on the basis of a p ,n transition are 30%͑Ref.20͒and 43%.15We have seen above that the former corresponds to approximately twice as many irreversible processes asre-parison between the efficiency of solar energy reemissions and efficiencies of conversion of solar heat into work.Line AB —the efficiency U of the reemissions,according to Eq.͑1͒.Lines KB ,CEB ,and CDB show the limiting efficiencies of work performed in parallel with the solar energy reemissions.The line KB includes the efficiency of a Carnot cycle only for the radiation ͓Eq.͑3͔͒,line CEB —only for matter ͓Eq.͑2͔͒,line CDB —for matter and radiation inparallel.parison between the efficiencies of some reversible and irre-versible conversions of solar heat into work.Line LB —the limiting effi-ciency L of the reversible process without a Carnot cycle according to Eq.͑5͒.Lines KB ,CDB ,and CEB are the limiting efficiencies of Carnot cycles as reversible processes occurring in parallel with irreversible solar energy reemissions.Points a ,b ,c illustrate the proposed method for calculating the relative contributions of reversible processes in a generally irreversible con-version of solar heat into work.versible ones.From this point of view,Fig.5makes it pos-sible to consider interzonal transitions as reversible and irre-versible processes.IV.ANTENNA STATES OF THE ABSORBER PARTICLESLet us consider these particle states in a radiant energy absorber,where the transitions between them result from the absorption of photons.The states of atomic particles or their groups,as well as energy transitions between them,are said to be“working”if they take part in performing work.Figures 4and5show that solar energy conversions are not always working processes.The states of atomic particles and the energy transitions between them are said to be“antenna”ones if they take part in the absorption and emission of ra-diant energy without the performance of work.Carnot cycles are examples of working processes,while cycles described below involving the photon reemission are examples of an-tenna processes.It is clear that antenna and working states are equilib-rium ones if cycles of the radiant energy conversion are not accompanied by entropy production,i.e.,if it takes place along line LB in Fig.5with an efficiency ofL from Eq.͑5͒.Let us take their total amount to be100%.Now,assume that the conversion of radiant energy consists of reversible work-ing processes with an efficiency of0from Eq.͑2͒and irre-versible antenna processes with an efficiency ofU from Eq.͑1͒,i.e.,that it corresponds to line CE.In this case,thecoordinates of all points divided byL is equal to the shareof working states,while1−/L is equal to the share ofantenna states.Figure5shows that the number of antenna states͑i.e., the number of nonequilibrium states͒decreases as the effi-ciencygrows along line CE.The isothermal growth of the efficiencyimplies that certain antenna states have become equilibrium states and that reversible transitions that do not generate work have appeared.If the temperature dependence ofis determined by experiment,wefind that Fig.5be-comes an effective means of interpreting and modeling the paths along which work is performed by solar cells.We will give a description of concrete examples in a subsequent work.It will make use of the general information about the antenna and working states of the absorber particles that is presented below.V.DISCUSSION OF RESULTSHistorical survey of works dealing with the thermody-namic limiting efficiencies of solar cells is given in Ref.4. These works focus on the efficiencies of the conversion of solar heat into work by a reversible process͑L from Eq.͑5͒͒and by Carnot cycles͓0andC from Eqs.͑2͒and͑3͔͒. However,their authors did not include one detail:one and the same isotherm belongs to radiation and matter.The present article made use of this aspect of the conversion of solar energy in order to give a description of the photon reemissions with and without work production.A compari-son of its efficiency made it clear that it is impossible to overcome the30%threshold in solar cell efficiency without reversible reemission.Let the point a on the line CDB in Fig.5denote the conversion of solar energy with an efficiency of30%.This implies that,when T A=430K,the working states account for 32%of all the states of the particles in the absorber,i.e., there are two antenna states for each working one.By the definition of the line CDB,the working states make up a reversible process.Therefore,the remaining antenna states belong exclusively to irreversible processes.If one were to make reversible processes out of1/3of the antenna states, the efficiencywould increase from30%to50%͑point b on the isotherm in Fig.5͒.The same effect may be attained along the line CD if there is the same number of working and antenna states.However,the absorber would need to have a temperature of800K,which is technically unfeasible.Let us consider another example.The zone theory pro-poses mechanisms of converting solar energy into work with an efficiency ofϳ60%,which cannot be reached by an ab-sorber if it is heated along the lines CD and CE in Fig.5.14,16 Let us denote this value by the point c on the isotherm430K in Fig.5.It can be reached by an isothermal process only if ϳ50%of the antenna states constitute a reversible process.Thus,the30%efficiency threshold in solar energy con-version can be overcome only if solar energy is reemitted reversibly.In real apparatuses,antenna states can constitute a reversible process only in combination with irreversible ones.Let us therefore recall the definitions of these terms.The notion of the reversibility of a process relates to the second law of thermodynamics and is applicable to any ther-modynamic system,although we have not found it applied to quantum systems in physical literature.Let us recall that the transition of a system from equilibrium state1to equilibrium state2is called reversible͑i.e.,bidirectional͒if one can re-turn from state2to state1without making any changes in the surrounding environment,i.e.,without compensations. The transition of a system from state1to state2is said to be irreversible if it is impossible to return from state2to state1 without compensations.Let us now use these notions to de-scribe the equilibrium between a black body and radiation as a continuous and infinite series of quantum electronic transi-tions.According to this definition,the reemission͑1231͒will alter the frequency distribution of photons in the cavity if states2and3are energetically distinct.For this reason,such an antenna process is irreversible.The state of radiation will not change if the reemission͑12131͒or͑12321͒can take place.The resulting antenna process will be reversible if the equilibrium state of matter is not violated.Every quasi-static process is reversible and infinitely slow.Are the continuous series͑12131͒and͑12321͒quasi-static processes?For the sake of simplicity,let us approxi-mate the spatial arrangement of particles by a periodic chain of elements that has a length of2m and that is separated by2Å.The time period during which solar radiation will excite only thefirst particle in the chain,while subsequent particles are excited only by emitted photons,is equal to 10−4s if the lifetime of the excited state isϳ10−8s.The timeneeded for the wave to travel down the chain and excite only the last particle isϳ10−14s.Therefore,the energy exchange due to reemission in a chain of particles is an infinitely slow process in comparison with the diffusion of electromagnetic radiation in the chain.Even the multiple repetition of reemis-sion͑121͒by one particle during10−6s is a quasi-static pro-cess,for the time taken by such reemission exceeds by a factor of100the lifetime of the excited states.Thus,the sequences of antenna states of absorber par-ticles in solar cells can take the form of quasi-static pro-cesses.Their isolation out of the general process of the con-version of solar energy into work͑which is a nonequilibrium process on the whole͒does not contradict the laws of ther-modynamics.Let us call the reemission of solar energy during an an-tenna process retranslation,if the temperature of the radia-tion remains constant,and transformation,if the temperature of the radiation changes.The retranslation of radiation by a black body is,by definition,a reversible process.Transfor-mation can take place both in a reversible and irreversible way.Therefore,the efficiency of the work performed by a solar cell can be improved by increasing the share of antenna as well as working equilibrium states in an absorber.VI.CONCLUSIONThe present article proposes a way of overcoming the 30%threshold for solar cell efficiency.It is not directly linked to the improvement of existing types of devices or the search for the optimal physical and chemical properties of the materials.In contrast to previous works,we have drawn attention to the fact that the efficiency of a device is also influenced by processes which do not involve the perfor-mance of work,called antenna processes.Antenna processes take place during the conversion of solar heat into work.They can be divided into reversible and irreversible processes theoretically.We have shown that an-tenna processes can occur in a quasi-static fashion.Accord-ing to the maximum work principle,an increase in the share of quasi-static antenna processes should lead to the perfor-mance of additional work by other processes.The reversibil-ity diagram of the conversion of solar heat into work͑Fig.5͒shows that there are no theoretical prohibitions to attain an efficiency ofL equalϳ93%by the room temperatures.This diagram will be a useful means for the interpretation of the theoretical and experimental investigations of the intermedi-ate band devices and other solar cells which are underway.Let us leave the discussion of technological matters re-lating to the manipulation of antenna processes aside for the time being.We will devote a subsequent article to this sub-ject.Let us only remark here that the conversion of solar energy involving the participation of antenna moleculesfig-ures in the description of photosynthesis in biology.Every chlorophyll molecule in plant cells,which is a direct conver-tor of solar energy,is surrounded by a complex of250–400 pigment molecules.21The thermodynamic aspects of photo-synthesis in plants were studied in Refs.19and22,yet the idea of antenna for solar cells was not proposed.We hope that the notions of the antenna and working states of ab-sorber particles will make it possible to attain very high ef-ficiencies of the radiant energy convertors,especially in those cases when solar radiation is not powerful enough to make solar cells work efficiently yet suffices to drive photo-synthesis in plants.1A.Luque and A.Marti,“Theoretical Limits of Photovoltaic Conversion,”in Handbook of Photovoltaic Science and Engineering,edited by A.Lu-que and S.Hegedus͑Wiley,New York,2003͒.2V.Badesku,ndsberg,A.De V os,and B.Desoete,J.Appl.Phys. 89,2482͑2001͒.3A.De V os,ndsberg,P.Baruch,and J.E.Perrot,J.Appl.Phys.74, 3631͑1993͒.ndsberg and G.Tonge,J.Appl.Phys.51,3996,R1͑1980͒.5A.De V os,Am.J.Phys.53,570͑1985͒.6H.Leff,Am.J.Phys.55,602͑1987͒.ndsberg and H.Leff,J.Phys.A22,4019͑1989͒.8A.De V os,Endoreversible Thermodynamics of Solar Energy Conversion ͑Oxford University Press,Oxford,1992͒.9M.Rubin,Phys.Rev.A19,1272͑1979͒.10F.Curzon and B.Ahlborn,Am.J.Phys.43,22͑1975͒.11J.M.Gordon,Am.J.Phys.58,1136͑1990͒.12A.Rose,J.Appl.Phys.31,1640͑1960͒.13A.L.Fahrenbruch and R.H.Bube,Fundamentals of Solar Cells.Photo-voltaic Solar Energy Conversion͑Academic,New York,1983͒.14M.A.Green,Solar Cells͑The University of New South Wales,Kensing-ton,Australia,1992͒.15J.Werner,S.Kolodinski,and H.Queisser,Phys.Rev.Lett.72,3851͑1994͒.16A.Luque and A.Marti,Phys.Rev.Lett.78,5014͑1997͒.17J.Werner,J.Mattheis,and U.Rau,Thin Solid Films480–481,399͑2005͒.18T.Trupke,M.A.Green,and P.Wuerfel,J.Appl.Phys.92,4117͑2002͒. 19P.Wuerfel,Physik der Solarzellen,2nd ed.͑Spektrum Akademie Verlag, Leipzig,2000͒.20W.Shockley and H.Queisser,J.Appl.Phys.32,510͑1961͒.21P.H.Raven,R.F.Evert,and S.E.Eichhorn,Biology of Plants,6th ed.͑Worth Publishers,Inc.,New York,1999͒.ndsberg,Photochem.Photobiol.26,313͑1997͒.。
淤浆聚乙烯聚合工艺及催化剂的进展

近年来,我国聚乙烯产业保持快速发展势头,产量、消费量增速世界领先,是世界上最大的聚乙烯进口国。
2021年国内聚乙烯表观消费量达到3697.55万吨,产量达2289.84万吨,进口量1458.87万吨。
其中高密度聚乙烯(HDPE)表观消费量为1656.16万吨,产能1476万吨,占PE总产能的55.5%。
HDPE进口量为663.36万吨,占我国聚乙烯进口总量的45.5%。
HDPE的生产工艺有淤浆法、气相法和溶液法等,以淤浆法为主。
根据反应器形式的不同,淤浆工艺可分为釜式法和环管法。
釜式淤浆工艺主要有Mitsui公司的CX工艺和LyondellBasell公司的Hostalen/ACP工艺。
环管淤浆工艺主要有Chevron Phillips Chemical公司的MarTECH® ADL工艺和Ineos公司的Innovene S工艺[1]。
本文针对上述淤浆法HDPE生产工艺的现状、技术特点及催化剂应用情况进行了详细介绍,对HDPE技术的发展进行了展望。
1 釜式淤浆聚合工艺1.1 CX工艺CX工艺由日本Mitsui公司开发,于1958年工业化。
CX工艺是一种连续搅拌釜式反应器(CSTR)工艺(图1)[1]。
该工艺采用Ziegler-Natta催化剂,正己烷为聚合介质,丙烯或1-丁烯为共聚单体。
CX装置有两个聚合釜,可通过调节聚合釜的串、并联模式生产单峰或双峰聚乙烯,采用单一催化剂体系即可生产双峰聚乙烯等多种牌号的树脂[2]。
CX工艺以正己烷蒸发撤热为主,辅以水冷夹套等方式撤热。
其中70%的聚合反应热通过正己烷蒸发撤除。
这种方式限制了CX工艺单线产能的提高[3]。
因此,近年来,不少企业给装置增加了釜外循环撤热,使装置产能大幅提高[4]。
图1 CX工艺典型流程图(并联)CX工艺的催化剂主要有Mitsui公司的PZ[5]催化剂、RZ催化剂[6]和北京化工研究院的BCH、BCE系列催化剂[3,7,8]。
titsstatic单词

titsstatic单词The word "titsstatic" does not have any recognized meaning in the English language. It appears to be a combination of two separate words, "tits" and "static," which have distinct meanings on their own.From a linguistic perspective, the word "tits" is a colloquial term for female breasts. It is considered informal and somewhat vulgar. The use of such language can be offensive and disrespectful, particularly when used inappropriately or without consent.On the other hand, "static" refers to a lack of movement or change, often used to describe something thatis fixed or stable. In the context of technology, static can refer to a form of interference or disturbance in electronic signals.Combining these two words, "titsstatic," does not yield a clear or coherent meaning. It is likely a nonsensicalterm or a typographical error. It is important to exercise caution when using or interpreting words that may have offensive connotations or lack a clear definition.Understanding the meaning of words and their appropriate usage is essential for effective communication. It is crucial to choose words carefully, considering the context and the audience. Respectful and inclusive language promotes positive interactions and prevents misunderstandings.In conclusion, the word "titsstatic" does not have a recognized meaning in the English language. It is a combination of two words that have separate meanings but do not create a coherent concept when combined. Using appropriate language and being mindful of the impact of our words is essential for effective communication.。
相变界面稳定性概念

Kinetics of isothermal phase transformations above and belowthe peritectic temperature:Phase-field simulationsG.Boussinot *,E.A.Brener,D.E.TemkinInstitut fu ¨r Festko ¨rperforschung,Forschungszentrum Ju ¨lich,D-52425Ju ¨lich,Germany Received 5May 2009;received in revised form 9October 2009;accepted 13November 2009AbstractWe present phase-field simulations of isothermal phase transformations in the peritectic system below and above the peritectic tem-perature.The physical processes involved are of different natures,involving either a triple junction or a liquid-film-migration (LFM)mechanism.Below the peritectic temperature,one of the solid phases steadily grows along the other.Above the peritectic temperature the phase transformation proceeds via the LFM mechanism.To the best of our knowledge,this mechanism has not been discussed in the literature as a generic process of phase transitions in peritectic systems.In addition to the LFM process,we also simulate melting along the solid–solid interface.Finally,we make a simplified linear stability analysis of the liquid film,supporting our simulation results.Ó2009Acta Materialia Inc.Published by Elsevier Ltd.All rights reserved.Keywords:Peritectic phase diagram;Phase-field model;Triple junction;Liquid-film-migration1.IntroductionIndustrial alloys,such as steels,often exhibit peritectic phase diagrams.At the peritectic temperature T P ,two solid phases and the liquid phase are in a three-phase equilib-rium.In comparison with eutectic alloys (in which an equi-librium also exists between three phases),phase transitions in peritectic systems have been much less investigated.A typical experiment concerning alloys involves direc-tional solidification,in which the sample is pulled in a tem-perature gradient.The properties of the solidified alloy strongly depend on the microstructure left behind the solid-ification front.In peritectic systems,the directional solidi-fication exhibits a large variety of microstructures.For example,one can observe coupled growth (simultaneous growth of alternative lamellae of the two solid phases par-allel to the growth direction),discrete bands (alternative layers of the different solid phases perpendicular to the growth direction),island formation (one solid phaseembedded in a matrix of the other solid phase)or oscilla-tory regimes.A review of these solidification microstruc-tures can be found in Refs.[1,2].The variety of different scenarios is due to the peritectic phase diagram,in which the two solid phases play a differ-ent role.The peritectic temperature T P corresponds to an equilibrium between the peritectic phase ðc Þ,the primary phase ðd Þand the liquid phase ðL Þ—see Fig.1.Below the peritectic temperature ðT 0<T P Þ,and suffi-ciently above c c ,the system is in the two-phase region ðc þL Þof the phase diagram (see Fig.1),and the solid–liquid ðc þL Þequilibrium mixture is stable (with the liquid concentration c L c ðT 0Þand the solid concentration c c L ðT 0Þ).On the other hand,the system is also in the ðd þL Þtwo-phase region,but the ðd þL Þequilibrium mixture is meta-stable (with the liquid concentration c L d ðT 0Þand the solid concentration c d L ðT 0Þ).Above the peritectic temperature ðT 0>T P Þthe ðc þL Þmixture is metastable,while the ðd þL Þmixture is stable.Hence,depending on the temper-ature T 0,the phase transformation from a metastable equi-librium to a stable equilibrium takes the form:ðd þL Þ!ðc þL Þfor T 0<T P and ðc þL Þ!ðd þL Þfor1359-6454/$36.00Ó2009Acta Materialia Inc.Published by Elsevier Ltd.All rights reserved.doi:10.1016/j.actamat.2009.11.017*Corresponding author.E-mail address:guilaume.boussinot@ (G.Boussinot)./locate/actamatAvailable online at Acta Materialia 58(2010)1750–1760T0>T P.The magnitude of the driving force for these phase transformations can be represented by the dimen-sionless supersaturation D¼ðc L dÀc L cÞ=ðc cÀc dÞ.We note that D<0ðD>0Þfor T0<T PðT0>T PÞ.Because diffusion in solids is very slow,one can observe aðdþcÞmixture for long time when the sample is below T P,even if this is outside the solid–solid two-phase region.The large variety of directional solidification microstruc-tures in peritectic systems makes a general theory quite a challenging task.Indeed,since the system evolves with the temperature gradient,solidification can occur above or below the peritectic temperature.The existence of the temperature gradient excludes the presence of any solid phase far ahead of the solidification front,where the tem-perature is high,and the presence of any liquid phase far behind the front,where the temperature is low.Because of the change in the stability of the two solid–liquid equilib-ria when one crosses T P,the directional solidification pro-cess is of a complex nature.An isothermal process,which is more simple,can be understood as the limit of vanishing gradient in a directional process.In this paper we investigate isothermal transformations below and above the peritectic temperature.The composi-tion of the sample is varied throughout the wholeðdþLÞtwo-phase region(hypo-and hyperperitectic compositions) for temperatures below T P,and is close to the peritectic composition c c for temperatures above T P.Our purpose is tofind generic steady-state moving patterns.For this purpose we use the phase-field numerical method.The code used is the one developed in Ref.[3]for eutectic directional growth.Thus we had to adapt it to the isothermal process. The conversion to the peritectic system is straightforward since it only consists of changing the phase diagram.The phase-field method has proven its efficiency in reproducing the solidification microstructures of peritectic systems.In particular,it qualitatively reproduces the vari-ety of microstructures during directional solidification in peritectic systems[4,5].The phase-field method is based on continuousfields which locally represent the physical state of the system.They obey some evolution equations, and these equations are solved at each point of the compu-tational grid.When describing a classical solidification process of a pure material,one uses a temperaturefield and a phasefield.The value of the phasefield indicates whether the system is solid or liquid.At the solid–liquid interface,the phasefield varies smoothly(on afinite thick-ness)from the value for the liquid to the value for the solid. Taking the limit of vanishing interface thickness,one must recover the sharp interface description of the problem.The sharp interface description essentially consists of solving the diffusion equation inside domains with boundary con-ditions at the interfaces.This is the real physical approach when one considers a separation of length scales between interface thickness(usually of atomic scale)and micro-structure.As in eutectic systems,the transformations in peritectic systems involve two solid phases(c and d)in addition to the liquid ually,more than one phase field is defined in order to distinguish more than two phases.The triple junction is the point where the three phases meet.In the sharp interface approach,one usually assumes thermodynamic equilibrium at the triple junction,leading to Young’s law.The latter states that the sum of the forces due to surface tensions acting on the triple junction van-ishes.Once surface tensions are known,the orientations of two interfaces with respect to the third one are deter-mined.Unlike the sharp interface approach,the triple junc-tion is not a special point in the phase-field model since one solves the same evolution equations of thefields at each grid point.However,in the limit,where the thickness of the interfaces vanishes,the phase-field model description of the triple junction should satisfy Young’s law,which is a non-trivial task[3].The possible process in peritectic systems which involves the motion of the triple junction is schematically represented in Fig.2a in the spirit of Ref.[6].Another process can take place without the triple junction(Fig.2b).Liquid-film-migration(LFM)is where a solidification front and a melting front move together separated by a liquid layer.The kinetics are then controlled by the diffusion in the liquidfilm.In the following we present our phase-field simulations of isothermal transformations in a model peritectic system above and below the peritectic temperature.In Section2, we present briefly the phase-field model(for more details, see Ref.[3]).In Section3,we present our simulation results in the case of T0<T P.The process,which involves a triple junction,occurs via a steady-state motion where the stable c phase grows along the metastable d phase.ThistransitionG.Boussinot et al./Acta Materialia58(2010)1750–17601751is known as the“peritectic reaction”.The configuration of the interfaces near the triple junction was suggested by Kurz and Fischer[6]and numerically reproduced by Nes-tler and Wheeler[7].We investigate the steady-state veloc-ity dependence of peritectic reaction on average concentration and temperature.We would also like to mention the work of Das et al.[8,9]on the peritectic trans-formation d!c occurring through solid diffusion as the next stage of the microstructure evolution after the peritec-tic reaction.In Section4,we present our numerical results above the peritectic temperatureðT0>T PÞ.The phase transforma-tion proceeds via a LFM mechanism.To the best of our knowledge,this mechanism has not been discussed in the literature as a generic process of phase transitions in peri-tectic systems.We investigate the temperature dependence of the steady-state velocity of the LFM.Moreover,in the case of T0>T P,we numerically investigate the melting of peritectic alloys along a c=d interface,and study the tem-perature dependence of the steady-state velocity.In Section 5,we briefly discuss the stability of the LFM process using a simplified linear analysis,and then give an explanation for the qualitative difference of physical processes involved in the cases of T0<T P and T0>T P.Finally,we make a brief comment on directional solidification.2.ModelThe free-boundary problem corresponding to the phase transformation in the peritectic system consists of the determination of the pattern produced by the different phases(i.e.the location of the interfaces between phases) and the concentrationfield.The concentrationfield c obeys the diffusion equation in the liquid with diffusion coefficient D:@c@t¼D$2c;ð1Þand we neglect diffusion in the solid phases.This may not be appropriate for alloys with high T P.However,we have verified that the introduction of a solid bulk diffusion coef-ficient D s%D=10does not alter qualitatively the results of the present paper.Furthermore,since we investigate gener-ic patterns for small deviations from peritectic equilibrium, we used a temperature-independent diffusion coefficient D.The mass balance equation at an interface between phase i and phase j reads:V nðc iÀc jÞ¼ÀD i@c=@n ji þD j@c=@n jj;ð2Þwhere V n is the normal velocity,and n is the vector normal to the interface.c iðjÞis the concentration of phase i(j)at the interface,D iðjÞis the diffusion coefficient in phase i(j),andthe normal gradient@c=@n jiðjÞis evaluated on the i(j)sideof the interface.If one neglects diffusion in cðdÞsolid phaseðD s=D!0Þ, Eq.(2)reads:V nðc L cðdÞÀc cðdÞLÞ¼ÀD@c=@n jL ;ð3Þat a cðdÞ=L solidification front where theflux of materialfrom the solid into the liquid can be neglected.For acðdÞ=L melting front,the situation is different.The concen-tration of the cðdÞsolid phase at the interface c cðdÞL is differ-ent from the bulk concentration in the cðdÞmetastablephase c1.In the limit D s=D!0(i.e.the diffusion lengthin the solid is smaller than the other length scales of theproblem),theflux of material from the cðdÞphase intothe liquid phase becomes independent of D s(see e.g.Ref.[10]for more details)and cannot be neglected:ÀD s@c=@n jcðdÞ¼V nðc cðdÞLÀc1Þ:For the cðdÞ=L melting front,Eq.(2)thus reads:V nðc L cðdÞÀc1Þ¼ÀD@c=@n jL:ð4ÞThe liquid concentration at the solid–liquid interface isc¼c L cðdÞÀðc cÀc dÞd cðdÞj:ð5ÞThis corresponds to the local equilibrium at a given tem-perature and takes into account the Gibbs–Thomson cor-rection due to the local curvature j of the interface,which is assumed to be positive for a convex solid–liquidinterface.The capillary lengths are given by:d cðdÞ¼r cðdÞðc PÀc cðdÞÞðc cÀc dÞf00Lðc PÞ;ð6Þwhere r cðdÞis the surface energy of the L=cðdÞinterface,f LðcÞis the free energy density of the liquid phase,andf00LðcÞis its second derivative with respect to c.We use the phase-field method to solve this problem.The model was developed in Ref.[3]to study eutectic direc-tional growth.Two different solid phases and one liquidphase should be described.Three phasefields are thendefined:piðrÞ;i¼c;d;L.The value of p iðrÞis1whenthe system is in phase i at the point r,and0otherwise.Thenpiis different from1and0only within a transition regionof width W,i.e.at the interfaces between different phases.The interface thickness W is arbitrary,and should not belinked to any physical length scale within the spirit of thephase-field method.Here,the surface tensions are assumedisotropic for the sake of simplicity.We have an additionalconstraint:pcðrÞþp dðrÞþp LðrÞ¼1;ð7Þwhich allows the evolution equations to be solved only fortwo phasefields.In addition to these two phasefields,onealso introduces a concentrationfield.We refer to Eqs.(4.1)and(4.2)in Ref.[3]for the time evolution of the phasefields and the concentrationfield.In the diffusion equation,the diffusion coefficient is chosen proportional to the liquidphasefield pLðrÞ.This allows Eq.(1)to be recovered in thelimit of vanishing interface width W,but is not the onlychoice.The theoretical two-phase equilibrium profile for thephasefields is the well-known hyperbolic tangent with acharacteristic length scale W.This theoretical profile isreached numerically by increasing W.If W is too small,1752G.Boussinot et al./Acta Materialia58(2010)1750–1760the small number of grid points within an interface leads to numerical problems.Since W has also to be much smaller than the characteristic length scales of the microstructure, one has tofind a compromise in order to avoid a prohibi-tive CPU calculation time.In the model for directional solidification,the calcula-tion proceeds through a regular shifting procedure of the simulation box in order to reproduce the pulling of the sample with constant velocity in the temperature gradient. The simulation box then represents the system in the frame in which the temperature is constant in time.In our case, the velocity is not imposed,and we should calculate it. Thus,we also use a shifting procedure,but one that is not regular.We locate a point of interest(e.g.the tip of a solidification front),and when this point has advanced by a certain small amount of grid points n,we shift the simu-lation box.This point of interest then stays approximately at the same position in the simulation box during the calcu-lation.If n0is the number of time steps needed to go through the n grid points,we calculate the velocity by V¼ðn D xÞ=ðn0D tÞ,where D x is the grid step and D t is the time step.When a steady state is reached,the interfaces and the concentrationfield are time independent in the sim-ulation box(in the frame moving with the steady-state velocity).They then move with the steady-state velocity in the laboratory frame.At the lateral sides of the simula-tion box,we impose zero normal derivatives for the phase fields and the concentrationfield.The physical parameters used in our calculations are the ones given in Table1of Ref.[11].They were used to model an Fe–Ni alloy.In Fig.3,we present the corresponding phase diagram.The horizontal axis is the dimensionless concentration~c¼ðcÀc PÞ=ðc cÀc dÞ,and the vertical axis represents the dimensionless supersaturation D¼ðc L dÀc L cÞ=ðc cÀc dÞ.The dimensionless equilibrium concentra-tions at the peritectic temperature are~c c¼À1:16and ~c d¼À2:16(and0for the liquid phase).The liquidus and solidus lines are considered parallel for each two-phase region.The liquidus lines are given by~c L cðDÞ¼À1:19Dand~c L dðDÞ¼À0:19D.In Fig.3theðcþdÞtwo-phase region is represented by vertical lines.A realistic descrip-tion is not relevant,since no diffusion is allowed in the bulk solid phases and theðcþdÞequilibrium does not enter the physical model described by Eqs.(1),(3)and(4).In the phase-field model,the capillary lengths given by Eq.(6)are not chosen independently(see Ref.[3]for more details).However,the average d0¼ðd cþd dÞ=2is chosen. Hence,we calculated the average of the capillary lengths given in Ref.[11]and took it as an input for our simula-tions.The interface energies determine the contact angles at the triple junction through Young’s law.In the phase-field model used here,these energies are considered equal for all interfaces.Thus,the interfaces at a triple junction divide the circle into three equal angles.Finally,the diffu-sion coefficient D gives the time scale of the physical pro-cess,and again,the value is taken from Ref.[11].To verify that the phase-field model is appropriate to study peritectic systems,we reproduced directional solidifi-cation processes for which some results of coupled growth obtained from a boundary-integral-method are given in Refs.[11,12].In Fig.4we present the steady-state patterns of coupled growth obtained from phase-field simulations which correspond to one period of the lamellar structure. The lamellae spacing(horizontal width of the presentedfig-ure)corresponds to k¼205d0for Fig.4a and k¼102:5d0 for Fig.4b.In both cases,the dimensionless concentration in the liquid far ahead of the solidification front is ~c¼À1:52.The thermal lengths,as defined in Ref.[11], areðl Tc¼224d0;l Td¼1391:5d0Þfor Fig.4a,andðl Tc¼37:5d0;l Td¼232d0Þfor Fig.4b.The interface width is in both cases,and for the rest of the present paper, W¼1:6d0.For Fig.4a the physical parameters are the same as in Ref.[11](Fig.9with G¼3K mmÀ1).For Fig.4b the physical parameters are the same as in Ref.[12](Fig.4.6).Wefind good agreement in the steady-state pattern obtained by the phase-field method and by the boundary-integral method.Moreover,this kind of micro-structure has been often reported in experiments in the Fe–Ni system[13],in the Ni–Al system[14],and more recently in the Cu–Sn system[15].However,a slight qualitative difference is present between our phase-field simulations and the boundary-integral results.In Fig.4a and b one can observe,at the tri-ple junction,the angle adopted by the c=d interface with respect to the direction of velocity.In comparison,in Fig.9in Ref.[11]and Fig.4.6in Ref.[12],the solid–solid interface remains straight(aligned with the velocity direc-tion)until the triple junction.In our phase-field calcula-tions the angle is a feature of the diffuseness of the interfaces.On the c=d side of the triple junction the liquidphasefield pLdecays smoothly,and so does the bulkdiffusion coefficient(which is proportional to pL).Some diffusion then occurs at the c=d interface in the vicinity of the triplejunction.G.Boussinot et al./Acta Materialia58(2010)1750–176017533.Transformation at T 0<T PIn the case T 0<T P the steady state obtained in our sim-ulations corresponds to the so-called peritectic reaction [16],where the stable c phase solidifies along the metastable d phase.This transformation involves a triple junction.A schematic illustration of the triple junction during this transformation is given in Ref.[6].The d phase melts,with an impurity flux towards the melting front,and the c phase solidifies,rejecting impurities.Far ahead of the triple junc-tion ðþ1Þ,the system consists of a ðd þL Þequilibrium,imposed as a boundary condition in our simulations.The d phase fraction is related to the average concentration by the lever rule.The most advanced point of c phase is used as the reference point for our shifting procedure.The width of the simulations is equal to k ¼102:5d 0,and the interface width is still W ¼1:6d 0.We have used several initial configurations to investigate the robustness of the obtained steady state,and one of these is displayed in Fig.5.It consists of a ðd þL Þequilibrium on the right side of the box (according to the lever rule)and a ðc þL Þequi-librium on the left side of the box.In between a liquid film of thickness k =4is left.The concentration in the liquid phase is c L c on the left side of the box (light grey)and c L d everywhere else (dark grey).Whatever the initial thicknessof the liquid film or phase arrangement on the left side of the box,the evolution of the simulation proceeds through a rapid shrinking of the liquid layer,followed by the growth of the c phase along the d phase.In Fig.6we show the steady-state pattern obtained for different average concentrations.The supersaturation is D ¼À0:07.The c phase (shown in white)grows to the right along the melting d phase (shown in black).The product of the transformation is a ðc þd þL Þmixture,except for the case of ~c ¼À1:9where the c front touches the channel wall and a ðc þd Þmixture is produced.In Fig.7we plot the steady-state dimensionless velocity Vd 0=D with respect to the dimensionless average concentration ~c .For small j ~c j the velocity shows weak variation,and decreases when j ~c j increases.For j ~c j ¼1:9where the c front touches the chan-nel wall,the velocity is significantly higher.In Fig.6we see that when the liquid phase fraction is large ð~c ¼À0:5;À0:7;À0:9;À1:2Þ,the c solidification front exhibits almost the same parabolic shape for any ~c .This shows that the influence of the channel boundary is negli-gible,and the c =L front can be interpreted as an Ivantsovparabola [17].We have checked that the observed PE´clet numbers qualitatively obey the Ivantsov relation.The dif-fusion field in the vicinity of the triple junction is almost independent of the average concentration,and the steady-state velocity is then weakly dependent on ~c (see Fig.7).When j ~c j increases,the diffusion field begins to be influ-enced by the channel boundary and the c =L front becomes flat behind the triple junction.The steady-state velocity then decreases.For the case ~c ¼À1:9,the velocity of the transformation ðd þL Þ!ðc þd Þis significantly higher than the one obtained for the transformation ðd þL Þ!ðc þd þL Þ.This suggests the presence of this solution in a certain range of average concentration,which could overlap with the one of the ðd þL Þ!ðc þd þL Þtransformations.Note that the comparison between the patterns obtained from phase-field simulations in Fig.6and theschematicFig.4.Pattern of the steady-state coupled growth during directional solidification simulated with the phase-field model.These simulations should be compared with Refs.[11,12](seetext).Fig.5.Initial configuration of the simulation for ~c ¼À0:7.The left (right)side of the box contains a c ðd Þphase fraction according to the lever rule.The concentration in the liquid (L )is c L c ðc L d Þon the left (right)side of the box.1754G.Boussinot et al./Acta Materialia 58(2010)1750–1760pattern in Fig.2a in the vicinity of the triple junction is irrelevant since the physical length scales are of the order of the interface width of the phase-field model.However,we verified that the phase-field simulations reproduce the typical pattern depicted in Fig.2a when the interface width is small compared to the length scales at the triple junction.Note also that,when we assume a small diffusion coeffi-cient in the solid phases,the c =d solid–solid interface becomes slightly inclined,which suggests that no d phaseexists at À1according to the peritectic transformation mentioned in Introduction and described in Refs.[8,9].3.1.Dependence on supersaturationWe have studied the dependence of the steady-state velocity on the supersaturation j D j (Fig.8).The calcula-tions were performed with a large liquid phase fraction in order to consider the case were the channel width has almost no influence on the velocity.This eliminates the channel width as an additional length scale in theproblem.Fig.6.Simulations at D ¼À0:07ðT 0<T P Þfor different average dimensionless concentrations ~c .The c phase (white)grows to the right along the d phase (black).The liquid is represented in grey.Left row from top to bottom:~c ¼À0:5;À0:7;À0:9;À1:2;right row from top to bottom:~c ¼À1:5;À1:7;À1:8;À1:9.G.Boussinot et al./Acta Materialia 58(2010)1750–17601755One observes that the velocity increases when j D j increases, which is expected.The curve approximately follows a j D j4 behavior.However,obtaining a scaling law from our phase-field simulations is a challenging task.Indeed,the range of investigated supersaturation is relatively small, and smaller supersaturations are unreachable due to the large characteristic length scales.4.Transformation at T0>T PWhen T0>T P the thermodynamic equilibrium consistsof aðdþLÞmixture.The metastable equilibrium consists of aðcþLÞmixture or a single c phase if the operating point lies outside of theðcþLÞtwo-phase region.The ini-tial configuration of the simulations is made of the metasta-ble equilibrium on the right side of the boxðþ1Þ,of the stable equilibrium on the left sideðÀ1Þ,and of a liquid film in between.In Fig.9,we present the initial configura-tion for D¼0:14and~c¼À1:15which lies in theðcþLÞtwo-phase region.The concentration in the liquid is set to c L c on the right side of the box(dark grey),to c L d every-where else(light grey).The channel width is equal to k¼205d0,with W¼1:6d0and a liquid layer with a width k=8.In Fig.10,we present the steady-state pattern corre-sponding to the initial configuration shown in Fig.9.The transformation consists inðcþLÞ!ðdþLÞand proceeds via an LFM process.In this case,the liquid layer between the two solid phases survives from the initial configuration until the steady state is reached.The LFM process is a physical mechanism often reported in experimental works on sintering[18]or partial melting[19].A schematic illustration is given in Fig.2b. LFM is related to the problem of grain-boundary migra-tion,where a thin intergranular liquidfilm can propagate during grain evolution[20].In Ref.[21]a description of LFM is made within the assumption of vanishing surface tension.A velocity selection theory was then developed [22],introducing the effect of surface tension(especially its anisotropy).Recently,a stability analysis of the liquid film in the presence of stress has been carried out[23]. The LFM process occurs via the co-operative motion of two fronts,one solidification front and one melting front, with a liquidfilm in between.The liquid concentration should be different at the two fronts(different equilibrium conditions),leading to a concentration gradient within the liquid necessary for a steady-state motion.Here,in the peritectic system(Fig.10),the d phase solidifies and the c phase melts,and the concentration gradient in the liquid is the consequence of the difference between c L d and c L c.We also obtained an LFM process when the average concentration is on the solidus line of theðcþLÞtwo-phase region.In Fig.11the corresponding steady-state pattern for D¼0:14is presented.The channel width is k¼102:5d0.4.1.Dependence on supersaturationIn order to save CPU time,we investigated the depen-dence of steady-state velocity on supersaturation for a metastable equilibrium comprising a single c phase,which allows smaller channel widths to be investigated.The variation of the dimensionless velocity Vd0=D with respect to D is shown in Fig.12.Within the investigated range of D the curve exhibits a weak non-linear behavior. In addition,we investigated the variation of the thickness h of the liquidfilm.As a characteristic value of h we take the distance between the d and c phase at the symmetry axis of the simulation.In Fig.12the variation of the dimension-less quantity Vh=D is also shown,and it exhibits a linear behavior.Within the investigated range of D the behavior of Vh=D corresponds to a variation of2%of h,while the velocity exhibits a variation of approximatively50%.The quasilinear behavior of the steady-state velocity can be understood using the balance equation where the concen-tration gradient is approximately proportional to D(since the liquidfilm thickness depends weakly on D).We focus now on the solution of the problem in free space.An Ivantsov solution exists[21],consisting of two confocal parabolas,one for the melting front and one for the solidification front.When making theappropriate Fig.9.Initial configuration for T0>T P with D¼0:14and~c¼À1:15.Fig.10.TransformationðcþLÞ!ðdþLÞcorresponding to the initialconfiguration shown in Fig.9.The velocity is indicated by the blackarrow.This transformation proceeds via a liquid-film-migrationprocess.Fig.11.Liquid-film-migration process for the transformation c!ðdþLÞwhere the metastable equilibrium consists of a single c phase,and theaverage concentration is exactly on the c solidus line.1756G.Boussinot et al./Acta Materialia58(2010)1750–1760。
Discrete and Global Symmetries in Particle Physics

1
Discrete Space-Time Symmetries
xµ → x′µ = Λµν xν
2
Lorentz transformations
(1)
2
preserve the invariance of the space-time interval
′µ xµ xµ = r2 − c2 t2 = r′ − c2 t′ = x′ . µx
2
R. D. Peccei
The pseudo-orthogonality of the Λ matrices detailed in Eq. (3) η = ΛT ηΛ allows the classification of Lorentz transformations depending on whether +1 ; −1
Discrete and Global Symmetries in Particle Physics
R. D. Peccei
Department of Physics and Astronomy, UCLA, Los Angeles, CA 90095-1547
arXiv:hep-ph/9807516v1 27 Jul 1998
(2)
This constrains the matrices Λµν to obey
ηµν = Λλµ ηλκ Λκν , where the matrix tensor ηµν is the diagonal matrix −1 1 . ηµν = 1 1
(3)
(4)
Discrete and Global Symmetries in Particle Physics
CST色散媒质说明

Material Parameters: default - DispersionSolve Materials New Material DispersionEdit Object Properties (navigation tree:Materials:material1Properties Dispersion)This is a dialog page of the Material Parameters dialog box.On this dialog page various dispersion models for the permittivity as well as for the permeability are available, representing different frequency dependent material formulations. Except for the magnetic gyrotropic behavior for biased ferrites, all models are also valid for an anisotropic material type.Please note that corresponding to common literature the input parameters distinguish between angular frequencies indicated by the unit "rad/s" (e.g., resonance frequencies) and normal frequency values in "Hz" (e.g., damping frequencies).Please see the Material Overview (HF) page for more detailed information about the different dispersion models.Dielectric dispersion frameDispersion model: Here, different dielectric dispersion models can be chosen, each definable by a different set of specific material properties.The first material parameter for all dielectric dispersions models is the epsilon infinity value, representing the high frequency limit of the permittivity.Debye 1st order: The first order Debye dispersion describes a material relaxation process, determined by the relaxation time and the epsilon static value.Debye 2nd order: The second order Debye dispersion describes a superposed relaxation process given by the summation of two separate first order Debye models. The corresponding parameters are the two relaxation times as well as both epsilon static values.Drude: The Drude dispersion model describes the dielectric behavior of plasma material, determined by the plasma frequency and the collision frequency representing damping effects.Lorentz:The Lorentz dispersion model describes a material resonance process, determined by the epsilon static value, the resonance frequency and the damping factor.Gyrotropic: The electric gyrotropic or so-called gyroelectric dispersion behavior is relevant for magnetized plasma media. The material parameters comprise the plasma frequency and the collision frequency as for the Drude dispersion. In addition, the cyclotron frequency and the biasing direction describe the effect of the homogeneous biasing field. Note that this material dispersion is not selectable for anisotropic material settings.General 1st order: For a detailed information, see Material Overview.General 2nd order: For a detailed information, see Material Overview.User: The dispersion fit is based either on a constant conductivity, general 1st order, or a general 2st order model. A list of eps' eps'' values can be defined by different frequency points by pressing the Dispersion List button.Magnetic dispersion frameDispersion model: Here, different magnetic dispersion models can be chosen, each definable by a different set of specific material properties.The first material parameter for all magnetic dispersions models is the mue infinity value, representing the high frequency limit of the permeability.Debye 1st order: The first order Debye dispersion describes a material relaxation process, determined by the relaxation time and the mue static value.Debye 2nd order: The second order Debye dispersion describes a superposed relaxation process given by the summation of two separate first order Debye models. The corresponding parameters are the two relaxation times as well as both mue static values.Drude: The description of this dispersion model corresponds to that of the dielectric material above. However, here this model offers just the possibility to define a specialized dispersion curve, the parameters plasma and collision frequency have no exact physical equivalence.Lorentz: The Lorentz dispersion model describes a material resonance process, determined by the mue static value, the resonance frequency and the damping factor.Gyrotropic: The magnetic gyrotropic or so-called gyromagnetic dispersion behavior is relevant for ferrite materials that are magnetized up to saturation by a homogeneous static magnetic field. The corresponding parameters can be defined either in the Gauss or SI unit system. In Gauss units, they are given by the Landé factor, saturation magnetization (4 Pi M), the resonance line width representing the damping effects and finally the external applied magnetic field vector (x,y,z). Using SI units as the input system instead, the parameters are given by the Larmor frequency, the gyrotropic frequency, the damping factor and finally the unit vector for the biasing direction (x,y,z). Note that this material dispersion is not selectable for anisotropic material settings.See the Material Overview for a description of inhomogeneously biased ferrites.General 1st order: For a detailed information see Material Overview.General 2nd order: For a detailed information see Material Overview.User: The dispersion fit is based either on a constant conductivity, general 1st order, or a general 2st order model. A list of mue' mue'' values can be defined by different frequency points by pressing the Dispersion List… button. Parameter conversion frameNote: This frame is only available for a selected magnetic gyrotropic dispersion model.System: The Gauss or SI unit system can be selected for different input parameters of the gyrotropic material.Frequency: Reference frequency for the conversion of material parameters given in Gauss units into the SI system.See alsoMaterial Parameters, Material Overview (HF), Change Material, Modeller View, Dielectric Dispersion Fit, Magnetic Dispersion FitMaterial Overview (HF)In CST MICROWAVE STUDIO®, several different material properties are considered to allow realistic modeling of practical simulation problems. The two basic materials available are PEC (P erfect E lectrically C onducting material) and Vacuum . Other more complex materials may be defined in the Material Parameters dialog. Each material is distinguished by its unique name and can be visualized in a selectable color and transparency.Considering linear behavior, in the frequency domain the dielectric and magnetic material parameters determine the ratio of the electric field and flux density and of the magnetic field and flux density, respectively:The material properties can be defined either as normal , describing isotropic media or with consideration of anisotropic behavior.In the following section, some special material declarations are discussed.Conducting materialsIntroducing material losses leads to complex permittivity or permeability values, respectively. This means that the material parameters have a real and an imaginary part, both frequency dependent. The losses are specified by the dielectric or magnetic loss angle or its corresponding tangent delta values:Consequently, the tangent delta value is given as the negative ratio between imaginary and real part of the complex permittivity or permeability, respectively:To realize an almost constant tangent value, or to set up a specific tangent delta curve, an internal dispersive first order Debye model (see below) will be fitted to the tangent delta input. The green curve on the right demonstrates the tangent delta dispersive behavior of such a model. Obviously, it is less frequency dependent than the conductivity model.Please note that no material exists in reality, providing a broadband perfectly-constant tangent delta value.Lossy metal: This material type simulates the penetration of electromagnetic fields inside a very good but not perfect electrically conductor by use of an internal one-dimensional surface impedance model. This offers the possibility to take the so-called skin effect into account without refining the mesh for these materials. However, please keep in mind that this model is physically reasonable only for a specific frequency range, defined by the solid's dimension and its material properties: the conductivity κ and the permeability μ . As mentioned above, on one hand the material has to represent a very good conductor, that means a material with a high conductivity value or, in other words, a material with a high tangent delta value:Obviously this defines an upper limit for valid frequencies, but on the other hand the frequency dependent skin depth and.andand.In general, every linear material behavior is described with help of the expressions above. Besides the special dispersivemodels explained later, differentpossibilities for loss definitions areavailable in CST MICROWAVE STUDIO®.One definition is the followingconductivity model :This model realizes a broadband constantconductivity, however, the correspondingtangent delta value is frequencydependent, as displayed in the rightpicture., .of the fieldshas to be smaller than the thickness d of the corresponding metal solid. This then defines a limit for the lowest applicable frequency:using a weight factor of approximately 0.2.Both constraints define together the valid frequency range for this material type, thus to take lower frequencies into account the material should be modeled applying a normal material type in connection with an electric conductivity.Consequently, for broadband simulations the frequency range should be split into two intervals.Note that this kind of material type is also available as a boundary condition to suppress unwanted box resonances of the structure model.Dispersive materialsTo consider frequency-dependent material behavior in broadband simulations, the most common models up to second-order dispersions are available. This includes relaxation and resonance effects as well as plasma or even gyrotropic media. In each case the microscopic material behavior is represented by a macroscopic description of the permittivity or permeability in the frequency domain. Hereby the static parameter limit is indicated by the subscript 's ' and correspondent to this the high frequency limit by infinity symbol.Relaxation process: The relaxation process, also called first-order Debye model is characterized by the following formulation for the relative permittivity, containing the relaxation time τ:The second-order Debye model is a superposition of two different first-order models sharing the same high frequency limit.Resonance process: The resonance behavior of a material is described by the Lorentz model , containing the resonance frequency ω0 and the damping factor δ:In the pictures above, the real and imaginary parts of the relative permittivity are shown. On the left a typical relaxation process is visualized by a Debye first-order dispersion model. The relaxation time determines the frequency range of significant changes. In contrast to this is a Lorentz resonance curve on the right, demonstrating the material resonance at the resonance frequency. Both models are also available for magnetic dispersions, i.e., for frequency dependent permeability.Cold plasma media: A special material behavior is given by cold plasma, also known as Drude dispersion. This Relaxation Process Resonance Processmodel describes the characteristics of an electrically conducting collective of free positive and negative charge carriers, where the thermic movement of electrons is neglected. Damping is obtained by the collision of the particles among each other, described with help of the collision frequency νc . Considering the specific plasma frequency ωp the correspondent relative permittivity is given asGeneral dispersion models: All dispersion models mentioned above can be described in form of a general polynomial formulation, either as a first or a second order model. In case of a dielectric dispersion the corresponding expressions are given asThe parameters of these two general models can be directly defined in the Dispersive Material Parameters dialog orby applying an automatic fitting scheme to a list of material data values in the Dielectric Dispersion Fit or Magnetic Dispersion Fit dialog.Biased plasma (electric gyrotropic) media:When a homogeneous magnetic biasing field is present in addition to the assumptions for the cold plasma, the effective material properties can be described by a gyrotropic permittivity tensor. If a z-directed biasing field is assumed, the correspondent permittivity tensor is given byHere, the plasma frequency is again ωp , and the cyclotron frequency ωb arises from the circular trajectory of electrons with charge e and mass m e in the constant biasing field B 0. Damping is again due to the collision of the particles among each other, as described by the collision frequency νc .Biased ferrite (magnetic gyrotropic) media:Applying a static magnetic field to ferrite material causes dispersive and anisotropic permeability parameters. In fact, this material behaves strongly non-reciprocally and is significant for many microwave applications like circulators or one-way transmission devices. Such materials are called magnetic gyrotropic or simply gyromagnetic and are described by the following permeability tensor, assuming a magnetization above saturation in the z -direction:Here, the Larmor frequency ωl = Γ μ0H 0 (with H 0 as the biasing static field) and the gyrotropic frequency ωm = Γ M Sare coupled to the magnetic flux density and saturation magnetization by use of the gyromagnetic ratio Γ = (g e ) / (2 m e). This factor is calculated from the Landé factor g in connection with the charge and mass value of an electron.Beyond it the damping factor α is determined by the resonance line width ΔH as.Note that this material description is given in SI units. However, the ferrite parameters often appear in Gauss units referring to the input parameters Landé factor , saturation magnetization and resonance line width . Thus, for convenience, the Dispersive Material Parameters dialog offers both possibilities.For Time-Domain solver runs, a homogeneous biasing field can be assigned to each material.Inhomogeneously biased ferrite media:and,respectively.with the elements andand finallywhere. with the elementsandFor cases where the inhomogeneity of the biasing field needs to be considered, the Frequency Domain Solver with tetrahedral mesh features a convenient way to automatically calculate the magnetostatic field before the high frequency solver run. The solver then applies this biasing field to determine the ; varying material properties of the ferrites. Set up low and high frequency materials and sources in a single model, and then activate the Calculate static B-field for Ferrites option in the Special Frequency Domain Solver Parameters, and start the solver.The material properties specified in the Dispersive Material Parameters dialog influence the initial mesh even if the magnetic field vector or the biasing direction and the Larmor frequency are overwritten with the corresponding values for the local magnetostatic field.It is recommended to enter the material properties in the Gauss system, where the Landé factor can be specified directly. The C; frequency is proportional to the biasing field's magnitude, with the factor containing the Landé factor.The latter is then assumed to be equal to two if the material properties are given in the SI system (which approximately holds for many ferrites.)Corrugated wallsCorrugated wall surface impedance models are only available for the general purpose frequency domain solver with tetrahedral mesh. The surface impedance is given bywith the gap width w, the tooth width t (which should be less than one tenth of the gap width), and the corrugation depth d, which must be much larger than the corrugation width for the model to be valid. The number of corrugations per wavelength should be large, with ten per wavelength being the lower limit. There is a resonance in the effective material behavior when the corrugation depth is close to a quarter wavelength.Note: The validity of the corrugated wall surface impedance model for a given simulation cannot be checked by the solver. Therefore, it is mandatory to compare the results obtained with the equivalent material (i.e., the corrugated wall) to the full model at least once for a given problem type.Weight densityBesides the mentioned electric and magnetic material properties also the density value of a can be defined. This is necessary to perform SAR calculation as a postprocessing step.See alsoModeller View, Material Parameters。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
and Vsusy = 1 exp −ae−2κφ 4κ2 Ae2κφ + B + Ce−2κφ , (1.3)
Here g0 , g1 , Λ, a, A, B and C are constants and κ2 = 4πG is the D -dimensional gravitational constant. Eqns. (1.1)–(1.2) are somewhat more general than is demanded by string theory. However, if we set g0 = 1 we obtain the standard tree-level coupling between the dilaton and the electromagnetic field, while setting g1 = −1, Λ = (Dcrit − Deff ) /(3α′ ), in the Liouvilletype term (1.2) yields the case of a potential corresponding to a central charge deficit. The term (1.3), on the other hand, is the type of potential which arises in four dimensions from ¶ supersymmetry breaking via gaugino condensation in the hidden sector of the string theory [8]. Potentials of the form (1.2) and (1.3) have been widely studied in string cosmologies [9], but to date the only investigations of static spherically symmetric solutions involving such terms have been restricted to the case of uncharged solutions [10–14]. Maki and Shiraishi [15] have recently derived non-static Kastor-Traschen type [16] cosmological multi black hole solutions for the action (1.1)–(1.2). However, such solutions were only obtained for certain special values of the constants g0 , g1 and of the time-dependent coupling in the dilaton cosmological scale factor. In many respects the action (1.1) is still over-simplified because it neglects the possible contribution of additional scalar fields, such as the string moduli which correspond to the extra dimensions of spacetime after dimensional reduction. Static spherically symmetric solutions involving both moduli and a dilaton have been discussed recently by Cadoni and Mignemi [17], and by Cvetiˇ c and Tseytlin [18], but in the absence of a potential. The introduction of a potential greatly complicates the situation, however, as is well demonstrated by the case of the quadratic potential, where a complete integration proved impossible even numerically [7]. Given the inherent difficulties involved in studies of models with non-trivial potentials, the present paper is intended only as a first step: we will not study the problem posed by eqns. (1.1)–(1.3) in full, but will restrict ourselves solely to the case of a Liouville-type potential V = Vexp . It is our hope that a further refinement of the approach discussed here can be applied to the more difficult case when a supersymmetry-breaking potential of the type (1.3) is also included. We are of course most interested in the case D = 4, but will leave D arbitrary, (with the only requirement that D > 2), as this does not involve many extra complications. Furthermore, we will also leave the dilaton coupling to the electromagnetic field arbitrary, rather than immediately specialising to the string case (g0 = 1).
S.J. Poletti and D.L. t of Physics and Mathematical Physics, University of Adelaide, Adelaide, S.A. 5005, Australia.
ABSTRACT
We derive the global properties of static spherically symmetric solutions to the EinsteinMaxwell-dilaton system in the presence of an arbitrary exponential dilaton potential. We show that – with the exception of a pure cosmological constant ‘potential’ – no asymptotically flat, asymptotically de Sitter or asymptotically anti-de Sitter solutions exist in these models.
‡ A fascinating discussion of the observational consequences of a very weakly coupled massless dilaton is given in [5]. § Gregory and Harvey also considered a potential of the form V = 2m2 cosh2 φ. 2
⋆ E-mail: spoletti@.au † E-mail: dlw@.au 1
1. Introduction
There has been considerable interest recently in the properties of ‘stringy’ black holes: classical solutions of tree-level string effective actions, in which the Einstein action is supplemented by fields such as the axion, gauge fields, and the dilaton which couples in a non-trivial way to the other fields. In particular, dilaton black holes have been shown to have novel thermodynamic properties [1–3], and to behave like elementary particles in some scattering scenarios [4]. Unfortunately, much of the work on dilaton black holes to date has involved models with one serious deficiency: the dilaton has usually been assumed to be massless. It is widely believed, however, that the dilaton must aquire a mass through some symmetry breaking mechanism. Indeed, this is necessary in order to avoid long-range scalar forces which would ‡ otherwise arise . Gregory and Harvey [6] and Horne and Horowitz [7] have now finally made some investigation of black hole models which include a mass term – they have chosen a § standard quadratic potential for the dilaton field . While a rigorous proof of the existence of black hole solutions in these models has still to be given, the arguments of Horne and Horowitz [7] are nonetheless compelling. Ultimately, it would be physically desirable to investigate models of black holes in effective dilaton gravity theories in four dimensions which involve a dilaton potential generated by some specific symmetry breaking mechanism, rather than simply an ad hoc potential, as in the work to date [6,7]. In particular, one could consider an (Einstein frame) action such as S= √ dD x −g 1 1 R − g ab ∂a φ ∂b φ − V (φ) − exp 2 4κ D−2 4 −4g0 κφ D−2 Fab F ab , (1.1)
