The cotranslational function of ribosome-associated Hsp70 in eukaryotic protein homeostasis
Cohesion in English

Cohesion in EnglishThe various kinds of cohesion had been out lined by MAK Halliday in his writings on stylistics and the concept was developed by Ruqayia Hasan in her University of Edinburgh doctoral thesis.Cohesive relations are relations between two or more elements in a text that are independent of the structure: for example between a personal pronoun and an antecedent proper name, such as John ….he. A semantic relation of this kind may be set up either within a sentence with the consequence that when it crosses a sentence boundary it has the effect of making the two sentences cohere with one another.The major function of cohesion is text formation. As defined: text is a unified whole of linguistic items, this unity of text as a semantic whole is source for the concept of cohesion.So first we will explore the concept of text.TextText in linguistics refers to any passage spoken written of whatever length that forms a unified whole. A reader can easily identify whether the passage he is reading is a text or otherwise a collection of unrelated sentences. A text may be: spoken, written, prose, verse, dialogue, monologue, single proverb, a single cry for help or all day discussion on a committee. A text is a unit of language in use. A grammatical unit that is larger than a sentence. A text is not something that is like a sentence only bigger or larger. It is misleading. Rather text can be best defined as a semantic unit; a unit not of form but of meaning. If it is semantic unit, we will not expect to find it in structure of a sentence as a grammatical unit as phrase, clause etc.So text is not consist of sentence but it is realized by sentence or encoded in sentences.There are certain objective factors involved that constitute a text.Constituents of Text1.Texture:2.Ties:3.Cohesion1. Texture:Texture is that feature of text which made it a unified whole.According to ‘The Concise Oxford Dictionary of Linguistics by P.H. Mathews’ cohesion and coherence are sources which create texture. Cryst al adds ‘informativeness’ to cohesion and coherence.Example:Wash and core six cooking apples. Put them into a fireproof dish.Here them reference back to six cooking apples to create cohesion between the two sentences. Here we make a presupposition about the relationship between them and six cooking apples but it is not enough only to make a presupposition rather that presupposition must be satisfied to create texture as shown in the example . These two items are co referential and this coreferentiality creates texture.Here are five cohesive devices to create texture:(i)Reference(ii)Substitution(iii)Ellipses(iv)Conjunction(v)Lexical Cohesion2. TiesThe term refers to a single instance of cohesion.Example:Wash and core six cooking apples. Put them into a fireproof dish.Them and six cooking apples show reference as tie.If we take the Example:Wash and core six cooking apples. Put the apples into a fireproof dish.Here are two ties(i)Reference(ii)RepetitionCohesive analysis of text is made in terms of tie for a systematic account of its patterns which are a source for texture. Onward we will use the term ‘cohesive tie’ in place of ‘tie’.Here are five different kinds of cohesive ties that are also called cohesive devices:(i)Reference(ii)Substitution(iii)Ellipses(iv)Conjunction(v)Lexical cohesionMAKH and RH have based their model of cohesion on these cohesive ties. A detailed review is given here in the proceeding discussion.3. Cohesion‘The Concise Oxford Dictionary of Lin guistics by P.H. Mathews (1997)’ defines cohesion in term of syntactic unit (sentence).‘ A Dictionary Of Linguistics And Phonetics by David Crystal (1997)’ defines cohesion in terms of a grammatical unit (words)MAKH and RH (1976) argued that the concept of cohesion is semantic one. For them it refers to relation of meaning that:exists with in textgives the text texturedefines the text as textThis relation of meaning between the elements gives the reader presupposition. This is another way of approaching the notion of cohesion that presupposing and the presupposed give us a presupposition at semantic level as a relation of meaning: The one element presupposes the other i.e. the one element cannot be decoded without the presupposed.Example:Time fliesYou can’t; they fly too quickly.You can’t (Ellipses)They (Reference)Fly (Lexical Cohesion)Types OF CohesionLanguage is multiple coding system comprising three levels of coding:Meaning The semantic systemWording The lexicogrammatical system(grammar an vocabulary)Sounding/writing The phonological and orthographical systemCohesive relation fit into the overall pattern of language. Cohesion is expressed partly through vocabulary and partly through grammar.:1. Grammatical Cohesion(i) Reference(ii) Substitution(iii) Ellipses2. Lexical CohesionThe distinction between grammatical cohesion and Lexical cohesion is a matter of degree and MAHK RH suggested not to go in the depth of these overlapping areas and that conjunction is on the border line of the two types mainly it is grammatical but with the lexical component so we cannot clearly distinguish between the two types.Cohesion and the Linguistics StructureTexture and StructureStructure is one mean of expressing texture. Text consists of one sentence are fairly rare but they can be single sentences as well for ExampleNo smokingWonder never ceaseBut most of the text extends beyond the confines of single sentences so structure is important in a text as structural units such as phrase, clause and sentence which express the unity of text. But our use of term Cohesion refers especially to the non structural text forming relation. They are semantic relations and the text is a semantic unit.Cohesion With in the TextSince cohesive relation is not concerned with structure, they may be found just as well with in the sentence as between sentences cohesive relation are beyond the sentences boundaries. Cohesion is semantic relation between one element in the text and some other element that is crucial for its interpretation. This other element must also be found with in the text. Cohesion refers to the range of possibilities that exist for linking something with what has gone before.The Place of Cohesion in the Linguistics SystemHalliday (1985) has described three major functional semantic components:(i)The Ideational(ii)The Interpersonal(iii)The TextualTable: the place of cohesion in the description of English functional components of semantic systemConclusionCohesion is a part of text forming component in the linguistics system. It links together the elements that are structurally unrelated through the dependence of one on the other for its interpretation. Without cohesion the semantic system cannot be effectively activated at all. Cohesive Devices(i)Reference(ii)Substitution(iii)Ellipses(iv)Conjunction(v)Lexical cohesion1. ReferenceThere are certain items in any language which cannot be interpreted semantically in their own right rather they make reference to something else within the text for their interpretation. Here is an example of referenceDoctor Foster went to Gloucester in a shower of rainHe stepped in puddle right up to his middleAnd never went there againHere in the above exampleHe refers back to Doctor FosterThere refers back to GloucesterHe and there show that information about them is retrieved elsewhere within the text. It characterizes a particular type of cohesion which is called reference. The relationship of reference is on semantic level. The reference items must not match the grammatical item it refers to. What must match or the semantic properties of reference item in relation to the items it refers to.Reference can be sub-categorize as followReferenceExophora EndophoraAnaphora CataphoraExophoraIt indicates situational references. Anaphora signals that reference must be made to the context of situation. It is outside the text so it is called anaphoric reference.Example;For he’s a jolly good fellow and so say all of us.Here text is not indicating who he is?He can be recognized by the situation in which expression is used. They are not source of cohesion because there presupposition cannot be resolved within the text rather the presupposition is found outside the text.EndophoraIt is a general name for reference within the text. This reference can be of two types.(i)Anaphora: Reference back(ii)Cataphora: Reference forwardExample:Child: Why does that one come out?Parent: That whatChild: That one.Parent: That one what?Child: That lever there that you push to let the water out.That one that lever (cataphoric reference)That lever that one (anaphoric reference)Types of referenceThere are three types of reference(i)Personal Reference(ii)Demonstrative Reference(iii)Comparative ReferenceIt is better first explain the structure of nominal group then proceed towards three types of Reference. It is because we will analyze nominal group for cohesive analysis of these cohesive devices.Nominal GroupThe logical structure of the nominal group (noun phrase) is that it consists of head with optional modifier the modifying elements include some which precede the head and some which follow it. They can be referred as Pre modifier and Post modifier respectively.ExampleThe two high stone wall along the roadside.Wall ------------ HeadThe two high stone ------------ Pre modifierAlong the roadside ------------ Post modifierThe modifier can be further subcategorized as:(i)Deictic(ii)Numerative(iii)Epithet(iv)Classifier (v)Qualifier (vi) Thing ExampleTheir famous old red wine.Deictic Deictic epithet classifier thingDeterminer adjective adjective adjective nounI. Personal ReferenceIt is a reference by means of function into a speech situation through the category of the person in the form of personal pronouns. The category of persons includes the three classes of personal pronouns. The category of person includes the three classes of personal pronouns. During the communication process the speech roles are assigned to the participants through the person system as:SpeakerAddresseeIt/one are used as a generalized form for other itemsExampleIf the buyer wants to look the condition of the property, he has to have another survey. One carried out on his own behalf.Here in the above example the use of personal pronouns is a source of personal referenceBuyerhe hisSurvey oneIf possessive pronouns are used, they give two more notions other than Speaker and Addressee. They are that of Possessor and Possessed as I the following exampleThat new house is John’s. I didn’t know it was hisPossessor JohnPossessed house shown by the use of his and ‘sThe following table shows the system of person for personal reference.Table: Personal ReferenceSemantic category Grammatical functionClassPerson:speaker (only)addressee (s), with/withoutother person(s)speaker and other personother person, maleother person, femaleother persons, objectsobject; passage of textgeneralized personII. Demonstrative ReferenceIt is essentially a form of verbal pointing. The speaker identifies or points pout the referent by locating it on scale of proximity. The system of demonstrative pronoun is given in the following table.Table: Demonstrative ReferenceSemantic category Grammatical functionProximity: near far neutralExamplesLeave that there and come here .Where do you come from?I like the lions and I like the polar bears. These are my favorites and those are my favorites too.III. Comparative ReferenceHere two types of comparison are given:(i)General Comparison(ii)Particular Comparison(i)General ComparisonHere things compared show likeness or unlikeness without considering any particular property. Likeness or unlikeness is referential property as something is can be like something else.ExampleIt’s the sa me cat as the one we saw yesterday.Its different cat from the one we saw yesterday(ii)Particular ComparisonHere comparison is made on the scale of quantity or quality it is a matter of degree compare things on this scale. In other words we can say it expresses the comparability between things.ExampleTake some more teaWe are demanding higher living standard.There are twice as many people there as the last time.Table: Comparative referenceClassGeneral comparison:Identitygeneral similaritydifference (i.e. non-identity or similarity)Particular comparison:2. SubstitutionSubstitution is replacement of one linguistic item by another. Ellipses is also a kind of Substitution where one linguistic item is replaced by nothing/ zero. Therefore it is an omission of an item.When we talk about replacement of one item by another, we mean replacement of one word/phrase with another word or phrase. We can say substitution is a relation on lexicogrammatical level. It is used to avoid repetition of a particular item. But while locating cohesion through substitution semantic is involved.ExampleMy axe is too blunt. I mist get a sharper one.You know John already knows. I think everybody does.Substitution is confined to text so exophoric substitution is rare. Most of the substitutions are endophoric and that of anaphoric type. But cataphoric substitution can also found in certain circumstances.Types of SubstitutionThere are three types of substitution.(i)Nominal Substitution(ii)Verbal Substitution(iii)Clausal substitution1.Nominal SubstitutionThere are three nominal substitutes.one, ones, same.The nominal substitute one/onesThe substitute one/ones always function as head of a nominal group and can substitute only for an item which is itself head a nominal group.ExampleI’ve heard some strange stories in my time. But this one was perhaps the strangest one of all.Note: The word other than a substitute can be used as(i)The personal pronoun one(ii)Cardinal numeral one(iii)Determiner oneThe nominal substitute sameSame typically accompanied by the presuppose an entire nominal group.ExampleA:I’ll have two poache d eggs on toast, please.B: I’ll have the sameThe Same can have following expressions as:Say the sameDO the sameBe the same2. Verbal SubstitutionThe verbal substitute is do. This operates as head of a verbal group. Lexical verb is replaced by do and its position is on the final in the group.Example from AliceThe words did not come the same as they used to do.It can also substitute for a verb plus certain other elements in the clause.ExampleI don’t know the meaning of half those language words and what’s more, I don’t believe you do either.Note: The word Do other than as substituteLexical verb do (he is doing)General verb do (they did a dance)Pro-verb do {do(action), happen(event)}Clausal substitutionHere presupposed is not an element within the clause but an entire clause. So and Not are clausal substitutesExampleIs there going to be an earth quake? - it says soTypes of Clausal SubstitutionThere are three types of clausal substitution.Substitution of reported clauseSubstitution of conditional clauseSubstitution of modalized clause1. Substitution of Reported ClauseThe reported clausal that is substituted by so or not is always declarative whatever the mood of the presupposed clause is whether interrogative or imperative.ExampleHas everyone gone home? I hope not.I didn’t think so.(I hope not (that) every one has gone home)Is this mango ripe? – It seems so.The essential distinction to be made here is that between reports and facts. Reports can be substituted whereas facts can not, reason is that facts are encoded at semantic level while clausal substitute works at lexicogrammatical level only.2.Substitution of Conditional ClauseConditional clause are also substituted by so and not especially following if /assuming so / suppose so etc.ExampleEveryone seems to think he’s guilty. If so, no doubt he’ll offer to resignWe should recognize the place when we come to it. Yes, but supposing not then what do we do?3.Substitution of Modalized ClauseSo and not also occur as substitute for clauses expressing modality.Example‘May I give you a slice?’ she said.‘Certainly not’ the red queen said.。
《翻译的语言学派》

总 论
西方翻译的两大翻译学派——语言学派和文艺学派贯穿了整个西方翻译史。翻译的语言学派又被称作“翻译科学派”。1959年雅可布逊发表他的著名论文《翻译的语言观》开始到1972年结束。西方译论的一大特点即与语言学同步发展 。
精选课件
一、布拉格学派与雅可布逊
成立:1926年10月6日,布拉格语方学会(The Linguistic Circle of Prague)召开第一次会议,布拉格卡罗林大学的英语语言和文学教授维伦·马泰休斯宣布了该学会的成立,也标志着布拉格语言学派的诞生。布拉格语言学派是继索绪尔之后最有影响的学派,其突出的贡献是创建了音位学.由于强调语言的交际功能和语言成分的区分功能,所以又常被称作功能主义者或功能语法学派。
精选课件
(4)语意走失的四个方面: a.原文内容涉及到本国特有的自然环境、社会制度、文化习俗,译文意思就必然走失; b.每一种语言都有自己的证明音、语法、词汇体系和运用方式,各种语言对世界上各种事物和概念的分类方法也不同。各种语言的词句很难在文体、感情色彩、抽象程度、评价尺度等四个方面完全对应;
精选课件
卡的“等值”翻译理论的意义
(一)从某一侧面反映翻译的本质在于确立“等值”关系;(二)等值关系确立并非静态地而是动态地把握;(三)对于从翻译学角度探讨双语转换机制的建立具有借鉴作用;(四)区别了翻译和转换两个概念。
精选课件
纽马克简介
彼得·纽马克(Peter Newmark ,1916-)是英国著名的翻译理论家和翻译教育家。他后来提出了著名的“交际翻译”和“语义翻译”法,20世纪90年代又提出“关联翻译法”,标志着他的翻译理论渐趋系统和完善。 纽马克的作品:论文 《翻译问题探讨》《交际性和语义性翻译》《翻译理论和翻译技巧》《翻译理念经与方法的某些问题》《专业翻译教学》《著作:翻译问题探索》《翻译教程》《论翻译》《翻译短译》
Collagen-structure, function, and biosynthesis

Collagens—structure,function,and biosynthesisK.Gelse a ,E.Po ¨schl b ,T.Aigner a,*aCartilage Research,Department of Pathology,University of Erlangen-Nu ¨rnberg,Krankenhausstr.8-10,D-91054Erlangen,GermanybDepartment of Experimental Medicine I,University of Erlangen-Nu ¨rnberg,91054Erlangen,GermanyReceived 20January 2003;accepted 26August 2003AbstractTherepresents a complex alloy of variable members of diverse protein families defining structuralintegrity and various physiological functions.The most abundant family is the collagens with more than 20different collagen types identified so far.Collagens are centrally involved in the formation of fibrillar and microfibrillar networks of the extracellular matrix,basement membranes as well as other structures of the extracellular matrix.This review focuses on the distribution and function of various collagen types in different tissues.It introduces their basic structural subunits and points out major steps in the biosynthesis and supramolecular processing of fibrillar collagens as prototypical members of this protein family.A final outlook indicates the importance of different collagen types not only for the understanding of collagen-related diseases,but also as a basis for the therapeutical use of members of this protein family discussed in other chapters of this issue.D 2003Elsevier B.V .All rights reserved.Keywords:Collagen;Extracellular matrix;Fibrillogenesis;Connective tissueContents1.Collagens—general introduction .............................................15322.Collagens—the basic structural module..........................................15323.Distribution,structure,and function of different collagen types...............................15353.1.Collagen types I,II,III,V and XI—the fibril-forming collagens ...........................15353.2.Collagen types IX,XII,and XIV—The FACIT collagens...............................15373.3.Collagen type VI—a microfibrillar collagen.....................................15383.4.Collagen types X and VIII—short chain collagens..................................15383.5.Collagen type IV—the collagen of basement membranes...............................15384.Biosynthesis of collagens ................................................15404.1.Transcription and translation ............................................15404.2.Posttranslational modifications of collagen .....................................15404.3.Secretion of collagens...............................................15414.4.Extracellular processing and modification ......................................15410169-409X/$-see front matter D 2003Elsevier B.V .All rights reserved.doi:10.1016/j.addr.2003.08.002*Corresponding author.Tel.:+49-9131-8522857;fax:+49-9131-8524745.E-mail address:thomas.aigner@patho.imed.uni-erlangen.de (T.Aigner)/locate/addrAdvanced Drug Delivery Reviews 55(2003)1531–15465.Functions of collagens beyond biomechanics ......................................15426.Perspectives .....................................................1542Acknowledgements....................................................1543References........................................................1543gens were once considered to be a group of proteinswith a characteristic molecular structure with theirfibrillar structures contributing to the extracellularscaffolding.Thus,collagens are the major structuralelement of all connective tissues and are also foundin the interstitial tissue of virtually all parenchymal 2.Collagens—the basic structural module The name ‘‘collagen’’is used as a generic term for proteins forming a characteristic triple helix of three polypeptide chains and all members of the collagen family form these the K.Gelse et al./Advanced Drug Delivery Reviews 55(2003)1531–15461532Table 1Table showing the various collagen types as they belong to the major collagen families TypeMolecular compositionGenes (genomic localization)Tissue distribution Fibril-forming collagens I [a 1(I)]2a 2(I)COL1A1(17q21.31–q22)bone,COL1A2(7q22.1)II[a 1(II)]3COL2A1(12q13.11–q13.2)III [a 1(III)]3COL3A1(2q31)Va 1(V),a 2(V),a 3(V)COL5A1(9q34.2–q34.3)COL5A2(2q31)COL5A3(19p13.2)XI a 1(XI)a 2(XI)a 3(XI)COL11A1(1p21)COL11A2(6p21.3)COL11A3=COL2A1Basement membrane collagens IV [a 1(IV)]2a 2(IV);a 1–a 6COL4A1(13q34)basement membranesCOL4A2(13q34)COL4A3(2q36–q37)COL4A4(2q36–q37)COL4A5(Xq22.3)COL4A6(Xp22.3)Microfibrillar collagen VI a 1(VI),a 2(VI),a 3(VI)COL6A1(21q22.3)widespread:dermis,cartilage,placenta,lungs,vessel wall,COL6A2(21q22.3)intervertebral discCOL6A3(2q37)Anchoring fibrils VII [a 1(VII)]3COL7A1(3p21.3)skin,dermal–epidermal junctions;oral mucosa,cervix,Hexagonal network-forming collagens VIII [a 1(VIII)]2a 2(VIII)COL8A1(3q12–q13.1)endothelial cells,Descemet’s membrane COL8A2(1p34.3–p32.3)X [a 3(X)]3COL10A1(6q21–q22.3)hypertrophic cartilageF ACIT collagens IX a 1(IX)a 2(IX)a 3(IX)COL9A1(6q13)cartilage,vitreous humor,corneaCOL9A2(1p33–p32.2)XII [a 1(XII)]3COL12A1(6q12–q13)perichondrium,ligaments,tendonXIV [a 1(XIV)]3COL9A1(8q23)dermis,tendon,vessel wall,placenta,lungs,liver XIX [a 1(XIX)]3COL19A1(6q12–q14)human rhabdomyosarcomaXX [a 1(XX)]3corneal epithelium,embryonic skin,sternal cartilage,tendon XXI[a 1(XXI)]3COL21A1(6p12.3–11.2)blood vessel wallTransmembrane collagens XIII [a 1(XIII)]3COL13A1(10q22)epidermis,hair follicle,endomysium,intestine,chondrocytes,lungs,liver XVII [a 1(XVII)]3COL17A1(10q24.3)dermal–epidermal junctionsMultiplexins XV [a 1(XV)]3COL15A1(9q21–q22)fibroblasts,smooth muscle cells,kidney,pancreas,XVI [a 1(XVI)]3COL16A1(1p34)fibroblasts,amnion,keratinocytes XVIII [a 1(XVIII)]3COL18A1(21q22.3)lungs,liverGiven are the molecular composition,the genomic localization of the different chains as well as the basic tissue distribution.K.Gelse et al./Advanced Drug Delivery Reviews 55(2003)1531–15461533extracellular matrix although their size,function and tissue distribution vary considerably.So far,26ge-netically distinct collagen types have been described [4,7–11].Based on their structure and supramolecular orga-nization,they can be grouped into fibril-forming collagens,fibril-associated collagens (FACIT),net-work-forming collagens,anchoring fibrils,transmem-brane collagens,basement membrane collagens and others with unique functions (see Table 1).The different collagen types are characterized by considerable complexity and diversity in their struc-ture,their splice variants,the presence of additional,non-helical domains,their assembly and their func-tion.The most abundant and widespread family of collagens with about 90%of the total collagen is represented by the fibril-forming collagens.Types Itissues [4,12,13].Type IV collagens with a more flexible triple helix assemble into meshworks restrict-ed to basement membranes.The microfibrillar type VI collagen is highly disulfide cross-linked and contrib-utes to a network of beaded filaments interwoven with other collagen fibrils [14].F ibril-a ssociated c ollagens with i nterrupted t riplehelices (FACIT)such as types IX,XII,and XIV collagens associate as single mol-ecules with large collagen fibrils and presumably play a role in regulating the diameter of collagen fibrils [9].Types VIII and X collagens form hexagonal networks while others (XIII and XVII)even span cell membranes [15].Despite the rather high structural diversity among the different collagen types,all members of the collagen family have one characteristic feature:a right-handed triple helix composed of three a -chains (Fig.1)[7,16].These might be formed by three identical chains (homotrimers)as in collagens II,III,VII,VIII,X,and others or by two or more different chains (heterotrimers)as in collagen types I,IV ,V ,VI,IX,and XI.Each of the three a -chains within the molecule forms an extended left-handed helix with a pitch of 18amino acids per turn [17].The three chains,staggered by one residue relative to each other,are supercoiled around a central axis in a right-handed manner to form the triple helix [18].A structural prerequisite for the assembly into a triple helix is a glycine residue,the smallest amino acid,in every third position of the polypeptide chains resulting in a (Gly-X-Y)n repeat structure which characterizes the ‘‘col-lagenous’’domains of all collagens.The a -chains assemble around a central axis in a way that all glycine residues are positioned in the center of the triple helix,while the more bulky side chains of the other amino acids occupy the outer positions.This allows a close packaging along the central axis of the molecule.The X and Y position is often occupied by proline and hydroxyproline.Depending on the colla-gen type,specific proline and lysine residues areFig.1.Molecular structure of fibrillar collagens with the various subdomains as well as the cleavage sites for N-and C-procollagenases (shown is the type I collagen molecule).Whereas they are arranged in tendon in a parallel manner they show a rather network-like supramolecular arrangement in articular cartilage.K.Gelse et al./Advanced Drug Delivery Reviews 55(2003)1531–15461534modified by post-translational enzymatic hydroxyl-ation.The content of4-hydroxyproline is essential for the formation of intramolecular hydrogen bonds and contributes to the stability of the triple helical conformation.Some of the hydroxylysines are further modified by glycosylation.The length of the triple helical part varies considerably between different collagen types.The helix-forming(Gly-X-Y)repeat is the predominating motif in fibril-forming collagens (I,II,III)resulting in triple helical domains of300nm in length which corresponds to about1000amino acids[3,4].In other collagen types,these collagenous domains are much shorter or contain non-triple helical interruptions.Thus,collagen VI or X contains triple helices with about200or460amino acids,respec-tively[4].Although the triple helix is a key feature of all collagens and represents the major part in fibril-forming collagens,non-collagenous domains flanking the central helical part are also important structural components(Fig.1).Thus,the C-propeptide is thought to play a fundamental role in the initiation of triple helix formation,whereas the N-propeptide is thought to be involved in the regulation of primary fibril diameters[3].The short non-helical telopeptides of the processed collagen monomers(see Fig.1)are involved in the covalent cross-linking of the collagen molecules as well as linking to other molecular structures of the surrounding matrix[38].FACIT collagens are characterized by several non-collagenous domains interrupting the triple he-lices,which may function as hinge regions[19].In other collagens like collagens IV,VI,VII,VIII or X,non-collagenous domains are involved in net-work formation and aggregation.In contrast to the highly conserved structure of the triple helix,non-collagenous domains are characterized by a more structural and functional diversity among different collagen families and types.Interruptions of the triple helical structure may cause intramolecular flexibility and allow specific proteolytic cleavage. Native triple helices are characterized by their resistance to proteases such as pepsin,trypsin or chymotrypsin[20]and can only be degraded by different types of specific collagenases.Collagenase A(MMP-1)[21],the interstitial collagenase,is expressed by a large variety of cells and is thought to be centrally involved in tissue remodeling, e.g. during wound healing.MMP-8(collagenase B)is largely specific for neutrophil granulocytes[22]and, thus,thought to be mainly involved in tissue destruction during acute inflammatory processes. MMP-13(collagenase C)[23]is expressed by hypertrophic chondrocytes as well as osteoblasts and osteoclasts[24]and therefore most likely plays an important role in cartilage and bone remodeling. Many other matrix metalloproteinases are able to cleave the denatured collagen(‘‘gelatin’’).The de-tailed analysis of the interplay of MMPs as well as specific inhibitors will describe the reactivities in vivo as well as potential pharmaceutical options for intervention[25–27].3.Distribution,structure,and function of different collagen types3.1.Collagen types I,II,III,V and XI—the fibril-forming collagensThe classical fibril-forming collagens include col-lagen types I,II,III,V,and XI.These collagens are characterized by their ability to assemble into highly orientated supramolecular aggregates with a charac-teristic suprastructure,the typical quarter-staggered fibril-array with diameters between25and400nm (Fig.2).In the electron microscope,the fibrils are defined by a characteristic banding pattern with a periodicity of about70nm(called the D-period)based on a staggered arrangement of individual collagen monomers[28].Type I collagen is the most abundant and best studied collagen.It forms more than90%of the organic mass of bone and is the major collagen of tendons,skin,ligaments,cornea,and many intersti-tial connective tissues with the exception of very few tissues such as hyaline cartilage,brain,and vitreous body.The collagen type I triple helix is usually formed as a heterotrimer by two identical a1(I)-chains and one a2(I)-chain.The triple helical fibres are,in vivo,mostly incorporated into composite containing either type III collagen(in skin and reticular fibres)[29]or type V collagen(in bone, tendon,cornea)[30].In most organs and notably in tendons and fascia,type I collagen provides tensile stiffness and in bone,it defines considerable biome-chanical properties concerning load bearing,tensileK.Gelse et al./Advanced Drug Delivery Reviews55(2003)1531–15461535strength,and torsional stiffness in particular after calcification.The fibril-forming type II collagen is the charac-teristic and predominant component of hyaline carti-lage.It is,however,not specifically restricted to cartilage where it accounts for about80%of the total collagen content since it is also found in the vitreous body,the corneal epithelium,the notochord, the nucleus pulposus of intervertebral discs,and embryonic epithelial–mesenchymal transitions[4]. The triple helix of type II collagen is composed of three a1(II)-chains forming a homotrimeric molecule similar in size and biomechanical properties to that of type I collagen[31].Collagen fibrils in cartilage represent heterofibrils containing in addition to the dominant collagen II,also types XI and IX collagens which are supposed to limit the fibril diameter to about15–50nm[32]as well as other non-collage-nous pared to type I collagen,type II collagen chains show a higher content of hydroxy-lysine as well as glucosyl and galactosyl residuesmatrix of hyaline cartilageof the type II collagen pre-mRNA results in twoforms of the a1(II)-chains.In the splice variant IIB, Fig.2.(A)Schematic representation of the supramolecular assembly of the collagen fibrils in the characteristic quarter-staggered form.The monomers are300-nm long and40-nm gaps separate consecutive monomers causing the characteristic appearance of the collagen type I fibrils on the ultrastructural level.(B+C)Collagen type I(B)and II(C)fibrils as they are arranged in normal tendon(B)and articular cartilage(C). Whereas they are arranged in tendon in a parallel manner,they show a rather network-like supramolecular arrangement in articular cartilage.K.Gelse et al./Advanced Drug Delivery Reviews55(2003)1531–15461536theexonN-terminal propeptide is excluded,whereas it is retained in the IIA variant,the embryonic form found in prechondrogenic mesenchyme [33,34],osteo-phytes [35,36],perichondrium,vertebrae [33]and chondrogenic tumors [37].The switch from IIA to IIB suggests a role during developmental processes chains and is widely distributed in collagen I contain-ing tissues with the exception of bone [38].It is an important component of reticular fibres in the inter-stitial tissue of the lungs,liver,dermis,spleen,and vessels.This homotrimeric molecule also often con-tributes to mixed fibrils with type I collagen and is also abundant in elastic tissues [39].Types V and XI collagens are formed as hetero-trimers of three different a -chains (a 1,a 2,a 3).It is remarkable that the a 3-chain of type XI collagen is encoded by the same gene as the a 1-chain of type II collagen and only the extent of glycosylation and hydroxylation differs from a 1(II)[4].Although it is finally not sorted out,a combination between differ-ent types V and XI chains appears to exist in various tissues [40–43].Thus,types V and XI collagens form a subfamily within fibril-forming collagens,though they share similar biochemical properties and func-tions with other members of this family.As men-tioned before,type V collagen typically forms heterofibrils with types I and III collagens and contributes to the organic bone matrix,corneal stro-ma and the interstitial matrix of muscles,liver,lungs,and placenta [12].Type XI collagen codistributes largely in articular cartilage with type II collagen [4,13].The large amino-terminal non-collagenous domains of types V and XI collagens are processed only partially after secretion and their incorporation into the heterofibrils is thought to control their assembly,growth,and diameter [44].Since their triple helical domains are immunologically masked in tissues,they are thought to be located central in the fibrils rather than on their surface [12,45].Thus,type V collagen may function as a core structure of the fibrils with types I and III collagens polymerizing around this central axis.Analogous to this model,type XI collagen is supposed to form the core ofII heterofibrils [3].A high content of in the N-terminal domains of a 1(V)-and a 2(V)-chains,with 40%of the residues being O-sulfated,supports a strong interaction with the more basic triple helical part and is likely to stabilize the fibrillar complex [46].3.2.Collagen types IX,XII,and XIV—The F ACIT collagensThe collagen types IX,XII,XIV,XVI,XIX,and XX belong to the so-called F ibril-A ssociated C olla-gens with I nterrupted T riple helices (FACIT colla-gens).The structures of these collagens are characterized by ‘‘collagenous domains’’interrupted by short non-helical domains and the trimeric mole-cules are associated with the surfaces of various fibrils.Collagen type IX codistributes with type II colla-gen in cartilage and the vitreous body [4].The heterotrimeric molecule consists of three different a -chains (a 1(IX),a 2(IX),and a 3(IX))forming three triple helical segments flanked by four globular domains (NC1–NC4)[47].Type IX collagen mole-cules are located periodically along the surface of type II collagen fibrils in antiparallel direction [48].This interaction is stabilized by covalent lysine-derived cross-links to the N-telopeptide of type II collagen.A hinge region in the NC3domain provides flexibility in the molecule and allows the large and highly cationic globular N-terminal domain to reach out from the fibril where it presumably interacts with proteo-glycans or other matrix components [13,49].A chon-droitin-sulfate side chain is covalently linked to a serine residue of the a 2(IX)-chain in the NC3domain and the size may vary between tissues [50].It might be involved in the linkage of various collagen fibres as well as their interaction with molecules of the extracellular matrix.Additionally,collagen type XVI is found in hyaline cartilage and skin [51]and is associated with a subset of the collagen ‘‘type II fibers’’(Graessel,personal communication).Types XII and type XIV collagens are similar in structure and share sequence homologies to type IX collagen.Both molecules associate or colocalize with type I collagen in skin,perichondrium,periosteum,tendons,lung,liver,placenta,and vessel walls [4].The function of these collagens,as well as of collagen55(2003)1531–15461537types XIX [52]and XX [53],within the tissue is still poorly understood.3.3.Collagen type VI—a microfibrillar collagen Type VI collagen is an heterotrimer of three differ-ent a -chains (a 1,a 2,a 3)with short triple helical domains and rather extended globular termini [54,55].This is in particular true for the a 3-chain which is nearly as twice as long as the other chains due to a large N-and C-terminal globular domains.However,these extended domains are subject not only to alternative splicing,but also to extensive posttrans-lational processing,both within and outside the cell [56,57].The primary fibrils assemble already inside the cell to antiparallel,overlapping dimers,which then align in a parallel manner to form tetramers.Following secretion into the extracellular matrix,type VI collagen tetramers aggregate to filaments and form an indepen-dent microfibrillar network in virtually all connective tissues,except bone [14,57,58].Type VI collagen fibrils appear on the ultrastructural level as fine fila-ments,microfibrils or segments with faint crossband-ing of 110-nm periodicity [58–63],although not all fine filaments represent type VI collagen [64–68].3.4.Collagen types X and VIII—short chain collagens Types X and VIII collagens are structurally related short-chain collagens.Type X collagen is a charac-teristic component of hypertrophic cartilage in the fetal and juvenile growth plate,in ribs and vertebrae [7].It is a homotrimeric collagen with a large C-terminal and a short N-terminal domain and experi-ments in vitro are indicative for its assembly to hexagonal networks [69].The function of type X collagen is not completely resolved.A role in endo-chondral ossification and matrix calcification is dis-cussed.Thus,type X collagen is thought to be involved in the calcification process in the lower hypertrophic zone [69–72],a possibility supported by the restricted expression of type X collagen in thecalcified zone of adult articular cartilage [73,74]and its prevalence in the calcified chick egg shell [75].In fetal cartilage,type X collagen has been localized in fine filaments as well as associated with type II fibrils.[76].Mutations of the COL10A1gene are causative for the disease Schmid type metaphyseal chondrodysplasia (SMCD)impeding endochondral ossification in the metaphyseal growth plate.This leads to growth deficiency and skeletal deformities with short limbs [77].Type VIII collagen is very homologous to type X collagen in structure but shows a distinct distribution and may therefore have different functions [78].This network-forming collagen is produced by endothelial cells and assembles in hexagonal lattices,e.g.in the Descemet’s membrane in the cornea [79].3.5.Collagen type IV—the collagen of basement membranesType IV collagen is the most important structural component of basement membranes integrating lam-inins,nidogens and other components into the visible two-dimensional stable supramolecular ag-gregate.The structure of type IV collagen is characterized by three domains:the N-terminal 7S domain,a C-terminal globular domain (NC1),and the central triple helical part with short interruptions of the Gly-X-Y repeats resulting in a flexible triple helix.Six subunit chains have been identified yet,a 1(IV)–a 6(IV),associating into three distinct heterotrimeric molecules.The predominant form is represented by a 1(IV)2a 2(IV)heterotrimers forming the essential network in most embryonic and adult basement membranes.Specific dimeric interactions of the C-terminal NC1domains,cross-linking of four 7S domains as well as interactions of the triple helical domains,are fundamental for the stable network of collagen IV [80].The isoforms a 3(IV)–a 6(IV)show restricted,tissue-specific ex-pression patterns and are forming either an inde-pendent homotypic network of a 3(IV)a 4(IV)a 6(IV)Fig.3.Schematic representation of collagen synthesis starting form the nuclear transcription of the collagen genes,mRNA processing,ribosomal protein synthesis (translation)and post-translational modifications,secretion and the final steps of fibril formation.(SP:signal peptidase;GT:hydroxylysyl galactosyltransferase and galactosylhydroxylysyl glucosyltransferase;LH:lysyl hydroxylase;PH:prolyl hydroxylase;OTC:oligosaccharyl transferase complex;PDI:protein disulphide isomerase;PPI:peptidyl-prolyl cis -trans -isomerase;NP:procollagen N -proteinase;CP:procollagen C-proteinase;LO:lysyl oxidase;HSP47:heat shock protein 47,colligin1).K.Gelse et al./Advanced Drug Delivery Reviews 55(2003)1531–15461538K.Gelse et al./Advanced Drug Delivery Reviews55(2003)1531–15461539heterotrimers(kidney,lung)or a composite network of a5(IV)2a6(IV)/a1(IV)2a2(IV)molecules[81]. Mutations of the major isoform a1(IV)2a2(IV)are assumed to be embryonic lethal,but defects of the a5(IV),as well as a3(IV)or a4(IV)-chains are causative for various forms of Alport syndrome due to the importance of the a3a4a6heterotrimer for stability and function of glomerular and alveolar basement membranes[3].4.Biosynthesis of collagensThe biosynthesis of collagens starting with gene transcription of the genes within the nucleus to the aggregation of collagen heterotrimers into large fibrils is a complex multistep process(Fig.3).Since most of our knowledge of these mechanisms is based on fibril-forming collagens,this discussion will mostly focus on type I collagen.It is likely that the basic mecha-nisms of triple helix formation and processing will also apply for other collagen types.4.1.Transcription and translationThe regulation of the transcriptional activities of collagens depends largely on the cell type,but may also be controlled by numerous growth factors and cytokines(for review,see Ref.[38]).Thus,bone formation is stimulated,at least in the adult,by members of the TGF-h-superfamily as well as the insulin-like-growth factors.In other tissues,fibro-blast-growth-factors and many other agents are even more important.To discuss this in more detail is beyond the scope of this review and needs to be evaluated for all collagens and tissues separately.Most collagen genes revealed a complex exon–intron pattern,ranging from3to117exons,with the mRNAs of fibrillar collagens encoded by more than 50exons.Therefore,in many cases,different mRNA species could be detected,caused by multiple tran-scription initiation sites,alternative splicing of exons or combination of both.For example,in the cornea and the vitreous body,a shorter form of type IX collagen mRNA is generated by an additional start site between exons6and7[4].Alternative splicing has been reported for many collagen types and was first described for type II collagen.A longer form of type II collagen(COL2A)is expressed by chondro-progenitor cells and varies from a shorter form (COL2B)where exon2is excluded[33]and which is the main gene product of mature articular chon-drocytes.More recently,more than17different tran-scripts have been reported for type XIII collagen[82] and alternative splicing also generates heterogeneous transcription products for collagens VI,XI,XII[82–85].In addition to splicing,the pre-mRNA undergoes capping at the5V end and polyadenylation at the3V end and the mature mRNA is transported to the cytoplasm and translated at the rough endoplasmatic reticulum.Ribosome-bound mRNA is translated into prepro-collagen molecules which protrude into the lumen of the rough endoplasmatic reticulum with the help of a signal recognition domain recognized by the cor-responding receptors.4.2.Posttranslational modifications of collagenAfter removal of the signal peptide by a signal peptidase(Fig.3),the procollagen molecules undergo multiple steps of post-translational modifications.Hy-droxylation of proline and lysine residues catalyzed by prolyl3-hydroxylase,prolyl4-hydroxylase,and lysyl hydroxylase,respectively.All three enzymes require ferrous ions,2-oxoglutarate,molecular oxy-gen,and ascorbate as cofactors.In fibril-forming collagens,approximately50%of the proline residues contain a hydroxylgroup at position4and the extent of prolyl-hydroxylation is species-dependent.The organisms living at lower environmental temperatures show a lower extent of hydroxylation[86].The presence of4-hydroxyproline is essential for intramo-lecular hydrogen bonds and thus contributes to the thermal stability of the triple helical domain,and therefore also to the integrity of the monomer and collagen fibril.The function of3-hydroxyproline is not known[3].The extent of lysine hydroxylation also varies between tissues and collagen types[87]. Hydroxylysine residues are able to form stable inter-molecular cross-linking of collagen molecules in fibrils and additionally represent sites for the attach-ment of carbohydrates.Glucosyl-and galactosyl-residues are transferred to the hydroxyl groups of hydroxylysine;this is catalyzed by the enzymes hydroxylysyl galactosyltransferase and galactosylhy-droxylysyl-glucosyltransferase,respectively(Fig.3).K.Gelse et al./Advanced Drug Delivery Reviews55(2003)1531–1546 1540。
219401794_沙棘果多糖的理化特征及其体外抗氧化活性

赵志强,朱叙丞,冯真颖,等. 沙棘果多糖的理化特征及其体外抗氧化活性[J]. 食品工业科技,2023,44(13):30−38. doi:10.13386/j.issn1002-0306.2022070288ZHAO Zhiqiang, ZHU Xucheng, FENG Zhenying, et al. Physicochemical Characteristic and Antioxidant Activity in Vitro of Seabuckthorn Fruit Polysaccharide[J]. Science and Technology of Food Industry, 2023, 44(13): 30−38. (in Chinese with English abstract). doi: 10.13386/j.issn1002-0306.2022070288· 研究与探讨 ·沙棘果多糖的理化特征及其体外抗氧化活性赵志强1,朱叙丞1,冯真颖1,陈海婷1,余佳乐1,闫 笛1,宋松泉2,申迎宾1, *,唐翠芳2,*(1.广州大学生命科学学院,广东广州 510006;2.湘南学院南岭现代种业研究院,湖南郴州 423043)摘 要:本研究通过热水提取法从沙棘果实中提取并初步纯化得到一种精制多糖(Seabuckthorn Berry Polysaccha-ride ,SBP ),对其单糖组成、分子量组成、微观表征、热稳定性、流变学特性等理化特征进行表征,并采用四种体外模型评价其抗氧化活性。
结果表明:该多糖的主要组分是一种大分子的酸性多糖,分子量为37.82×104 Da ,由岩藻糖、鼠李糖、阿拉伯糖、半乳糖、葡萄糖、木糖、葡萄糖醛酸等单糖构成,其摩尔比为0.244:0.098:0.265:0.075:0.091:0.081:0.103,含有α、β型糖苷键以及羰基、羧基、醛基、羟基等官能团,具有三螺旋结构,微观结构呈片状。
ABSTRACT Simulating Large Random Boolean Networks

Res.Lett.Inf.Math.Sci.,2007,Vol.11,pp33-4333 Available online at /research/letters/Simulating Large Random Boolean NetworksK.A.Hawick,H.A.James and C.J.ScogingsInstitute of Information&Mathematical SciencesMassey University–AlbanyNorth Shore102-904,Auckland,New Zealandemail:{k.a.hawick,h.a.james,c.scogings}@ ABSTRACTThe Kauffman N-K,or random boolean network,model is an important tool for exploring the properties of large scale complex systems.There are computational challenges in simulating large networks with high connectivities.We describe some high-performance data structures and algorithms for implementing large-scale simulations of the random boolean network model using various storage types provided by the D programming language.We discuss the memory complexity of an optimised simulation code and present some measured properties of large networks.KEY WORDSrandom boolean network;time series analysis;high memory;simulation.1IntroductionThe classical random boolean network(RBN)model was introduced by Kauffman to represent genetic regulatory networks[10,11].This model is also commonly known as the N-K model or as Kauffman networks and consists of a network of N nodes,connected to K neighbours.Each node is decorated by a boolean state which is initialised randomly,and also a boolean function of K inputs,each of which is randomly assigned from one of the possible boolean functions of K inputs.The model is studied by evolving the nodes synchronously,based on the state of their inputs. It is known that a transition occurs at K=2and that for smaller K the system rapidly moves to an attractor regime[9,13]with nodes converging to afixed value.Above the transition the nodes are chaotic,changing their values with no discernible pattern.Kauffman networks have been widely studied[5]and a variety of software tools are available for investigating small-sized networks[6].The model can be thought of as an extension of the binary cellular automaton model of Wolfram[12],and indeed for K=1the model shows similar properties as can be seen infigure1.Figure1shows the time evolution of four random configurations of the classical random boolean network model,each with128nodes,connected with K=1,2,3,4.In the case K=1most nodes quickly become locked and no longer change.In the cases K=3and K=4the system remains chaotic.Case K=2is known to be at the critical edge of chaos[3].The model has important uses in the study of networks and recently there has been interest in studying scale-free Kauffman networks[1].Unlike thefixed-K normal Kauffman networks,a scale-free network has an exponential distribution of connectivities,so although most nodes may have a small connectivity,some can have large values.Due to the way N-K networks are constructed and stored there are several practical implementation problems associated with simulating large N-K networks.In this paper we explore these and investigate possible data structures and algorithms for managing such large scale simulations.In section2we outline the core algorithms for constructing the structural network and the random boolean functions.We give a number of code fragments in the D programming language[2] to illustrate these ideas.In section3we present some performance analysis results and discussFigure 1:Classic random boolean network with 128nodes (horizontally),evolved for 256time steps (top to bottom)from a random initialisation,for K =1,2,3,4(left to right).Red/white denotes sites of value 0/1and mauve/grey denotes 0/1sites that are changing respectively.some of the measured properties of large N -K networks in section 4.We offer some conclusions for the future simulation of large network models in section 5.2Simulation Algorithms and ExperimentsThe network is represented as an array of neighbour lists,and each of the N nodes is decorated by a boolean state and a random boolean function.The state is initialised randomly with 50percent probability of being a zero or a one.A random boolean function is assigned statically (i.e.once)to each node.The connectivity of the network itself can be stored in a number of ways.It is fundamentally a directed graph and a convenient storage structure makes use of the dynamic D arrays.Our neighbours[i][n]structure lists the n neighbours of the i ’th node.D arrays have the ‘length ’property which can be assigned a value to invoke an appropriate dynamic reallocation of memory (and copying of old values)as necessary.This structure is suited to fixed-K networks as we discuss here,or those with a distribution of K values,added dynamically.The algorithm for assigning the network neighbours array so that each node has exactly K inputs is shown below.While this algorithm avoids self-arcs it does allow multi-arc neighbours –i.e.the same node can be picked as an input more than once.int [][]neighbours ;neighbours .l e n g t h =N;for (int i =0;i <N;i ++){neighbours [i ].l e n g t h =K;for (int n=0;n <neighbours [i ].l e n g t h ;n++){int c h o i c e ;//av o id s e l f −a r c s do {c h o i c e =randomInt ()%N;}while (c h o i c e ==i );neighbours [i ][n ]=c h o i c e ;}}The type system of the D programming language is somewhat stronger than that of C/C++and is therefore helpful in developing less error-prone simulation codes.The critical data type required for the random boolean network simulation is that of a logical bit.The original D language proposal had a bit as a fundamental data type,but due to implementation difficulties that was unfortunately demoted.A bool in D is stored as a whole byte and is type-checked for boolean logical operations.An int is the usual way C and(old fashioned)C++programs implement a logical bit.The standard library accompanying D,called‘Phobos’,comes with a BitArray data structure that has appropriate implementations of the opIndex and opAssignIndex operators that make the conventional array indexing syntax with square brackets([])work as expected.The efficiency tradeoffs are obvious:an int or uint is the same size as the machine architec-ture register width and is the fundamental unit of addressing,albeit wasting31bits on a32-bit architecture;a bool stored as a byte wastes only7bits of storage but in a modern machine it may be almost as fast to address as a machine word.A packed data structure such as the BitArray is fully efficient as regards memory utilisation but requires extra addressing arithmetic and bit-shifting operations to insert/extract individual logical bit values.The code fragments below are written in terms of a type definition for bits t that can be one of the following type definitions:typedef int[]b i t s t;typedef bool[]b i t s t;typedef BitArray b i t s t;The performance consequences of using these different bit storage representations are discussed in section3.The random boolean functions of K-inputs are represented by a truth table.An example encoding of this for the simple case of K=1and K=2are shown in tables1and3.Each column of the table represents the complete specification of a possible boolean function of K inputs.Although modern programming languages such as D do support higher dimensional arrays,it would be inefficient to use a separate programming dimension‘[][][][]...’of the truth table for each of the K boolean inputs i n,so they are encoded as an integer k=i0×20+i1×21+ i3×22+...+i n×2n for n=0,1,2,...,K−1and implemented as://compose b i t s t v e c t o r t o a k−coding:u i n t b i t s t o k(b i t s t b i t s){int nBits=b i t s.l e n g t h;u i n t k=0;for(int n=0;n<nBits;n++)i f(b i t s[n])k+=powersOf2[b];return k;}We can also write the inverse function to decode an integer into its bits,which is implemented as://decompose k−coding t o nBits−l e n g t h//b i t s t v e c t o r:b i t s t k t o b i t s(u i n t k,int nBits){b i t s t b i t;b i t s.l e n g t h=nBits;for(int n=0;n<nBits;n++){b i t s[n]=(k&powersOf2[n])!=0;}return b i t s;}Input Outputi0o00o01o02o030010110011Table1:Truth table of the22K=22=4random boolean functions for the example case K=1.Input States Possible Boolean FunctionsK2K N BF=22K12424163825641665,5365324,294,967,29666418,446,744,073,709,551,616Table2:Exponential growth of the number of inputs states and number of possible boolean functions with connectivity K.Input Outputk i1i0o00o01o02o03o04o05o06o07o08o09o10o11o12o13o14o15 0000101010101010101 1010011001100110011 2100000111100001111 3110000000011111111 Table3:Truth table of the22K=24=16random boolean functions for the example case K=2.The K=2inputs i1and i0are encoded as an integer k which can index into the TT[j][k]to give the boolean output value o for the j’th boolean function’s truth table,where j=0,1,...22K.There are other syntactic ways to encode these functions in C/C++or D,but these forms are type-safe against any of the three D typedefs described above.Using these function primitives,it is easy to construct each column of the truth table usinga bit representation of j.TT[j][k]is the truth table for the j’th boolean function and the k’thencoded bit-vector of the input bits and is implemented as:for(int j=0;j<nBFuncs;j++){TT[j]=k t o b i t s(j,nBits);}The update algorithm for the random boolean network model of N nodes,numbered i= 0,1,2,...,N−1is then:1b i t s t newState,swapState,b i t s;2newState.l e n g t h=N;3b i t s.l e n g t h=maxK;4for(int s t e p=0;step<nSteps;s t e p++){5for(int i=0;i<N;i++){6for(int n=0;n<neighbours[i].l e n g t h;n++){7b i t s[n]=s t a t e[neighbours[i][n]];8}9newState[i]=10TT[bFunc[i]][b i t s t o k(b i t s)];11}12}This uses a synchronous update whereby a new state vector is built at each time step and all N nodes are updated effectively together at once through the use of the swapping of state arrays newState and state.As might be expected there are various sweeping effects and other artifacts that arise if a non-synchronous update algorithm is used[8].Our code is written in terms of an integer lookup bFunc[i]that specifies which of the possible boolean functions is assigned to node i.The number of inputs states grows as2K with the number of inputs K.Table2shows that the number of possible boolean functions N BF=22K grows very rapidly and that it is not feasible to sample them all in any realisable network simulated on affordable computers except for K smaller than around5.If the number of possible boolean functions(N BF=22K)is very much greater that the number of nodes(N)then it is more effective to assign a truth table entry to each node directly and index TT[i]directly.In this case,TT is set up accordingly and the algorithm lines9and10are replaced by the line:newState[i]=TT[i][b i t s t o k(b i t s)];We can encode our simulation to deal with K=5by using64-bit unsigned integers for calcu-lations involving powers of two.We can in fact extend this to K=6providing we accept we are not uniformly sampling all possible boolean functions.The D language has provision for a128-bit unsigned integer(the ucent),but this is not yet supported by available distributions[4,7].We have been able to obtain timings for our random boolean network simulation up to values of K=1,2,3,4,6with the proviso that although the data structures are correct and therefore the timings are correctly representative,the sample space of possible boolean function is truncated for K=6.3Performance Results and AnalysisFor most of the sizes of system reported here we can quite easily use simple integers or bytes for the states rather than packed bits,as memory is typically dominated by the storage space required to specify the random boolean function at each node.The truth tables for the random boolean functions occupy occupy22K storage elements.In general the number of nodes N in our network is less than the possible number of boolean functions which grows very rapidly with K.In particular for K=5,N BF=225=232which presents interesting problems for programs running on32-bit architectures.Phobos provides a BitArray structure which can conveniently be used to make packed bit representations of the state and TT ing the overloaded[]array indexing operators in the D programming language,the BitArray can be used as a direct code substitute for any other typed array representation for the bits.Memory is then no longer a concern,but the manipulation of the k indices as32-or64-bitfixed entities still poses a limitation on our code.Table4shows some timing analyses for the random boolean network simulation code on various platforms.As table4indicates,the use of the bool data type is fastest in most cases for“medium”memory utilisation.We carried out these timing experiments on a variety of platforms and generally for “normal”program memory utilisation of less than2GBytes the timings show a practical time savings using the direct single byte bool type.At the time of writing64-bit operating systems support is becoming available and does work,but does not necessarily have appropriate cache and page management sizes.In table5we show that for large systems that explore more than the31-bit signed-integer addressable range of memory(up to2GBytes)the timing reflects the architecture’s cache size andN K bit t Time RSIZE128,0001int[] 1.2 6.11bool[] 1.1 4.91Bitarray 2.9 4.7128,0002int[] 2.0 5.72bool[] 1.9 4.92BitArray 4.2 5.1128,0003bool[] 2.4 4.94bool[] 5.09.55bool[] 5.115.06bool[]7.023.01,280,0001bool[]16.143.42bool[]25.643.43bool[]30.843.44bool[]68.666.25bool[]66.91446bool[]86.82251,280,0001int[]31.850.92int[]47.250.93int[]62.050.94int[]122.280.05int[]121.83946int[]147.37171,280,0001BitArray30.941.02BitArray48.741.03BitArray64.941.04BitArray98.862.85BitArray109.981.46BitArray121.081.42,560,0001bool[]43.786.12bool[]67.786.13bool[]83.586.14bool[]183.51295bool[]171.72876bool[]219.8449Table4:Timing measurements of the random boolean network simulation code for256steps, using GNU gdc D Compiler Version0.23,with optimization level-O6set.Timing is measured in seconds on a2.66GHz64-bin Xeon with4GB of available RAM.RSIZE is the resident memory size,as reported by the Unix top utility,measured in MB.page memory management system rather than being directly indicative of the simulation algorithm. In these cases the packed storage BitArray shows a marked improvement and does allow us to usefully simulate large systems of up to N≈107network nodes.N K bit t Time RSIZE5,120,0001bool[]9.31712bool[]12.31713bool[]15.11714bool[]24.92545bool[]48.85756bool[]83.589810,240,0001bool[]24.43422bool[]31.03423bool[]38.63424bool[]61.65065bool[]172.41,1206bool[]3251,75020,480,0001bool[]65.06832bool[]78.06833bool[]97.16834bool[]165.91,0085bool[]737.6>2,0006bool[]1437>3,00020,480,0001BitArray63.06482BitArray77.96483BitArray92.46484BitArray168.49735BitArray306.11,2606BitArray325.71,260Table5:Timing measurements of the random boolean network simulation code for8steps, using GNU gdc D Compiler Version0.23,with optimization level-O6set.Timing is measured in seconds on a2.66GHz64-bin Xeon with4GB of available RAM.RSIZE is the resident memory size,as reported by the Unix top utility,measured in MB.We can compute the memory complexity of the simulation algorithm and verify that the plat-form is genuinely allocating the correct amounts of(packed-bits)memory by recording the resident memory size as reported by a systems monitoring tool such as the Unix top utility.The memory utilisation is shown in table6.In the case in which bit t is implemented as an int we have N+N+N.K+N.2K+N=4N+NK+2K and if K=2,we have10N words which requires51MBytes(assuming a4-byte machine word size).This is consistent with the RSIZE values reported in table4and5.4Some RBN PropertiesAs shown infigure1,the random boolean network model has a varying degree of autocorrelation in time.Nodes’states will lock tofixed values below a critical value of K and will remain highly chaotic above it.A simple measure of the regime is given by thefirst-order correlation moment, which can be simply measured by:int nSame=0;for(int i=0;i<N;i++)Variable Number of Data typeName Elementsstate N bit tnewState N bit tneighbours N×K intN void*TT N×2K bit tN void*Table6:Memory complexity of the random boolean network simulator code.For neighbours and TT it is necessary to consider the all pointers required to efficiently implement a two-dimensional array.i f(s t a t e[i]==newState[i])nSame++;Other moments of the auto-correlation function can be computed to obtain a time-series analysis that identifies the set of attractors present in the network.For a statistically meaningful analysis we need to consider how the system self-averages.Ideally we would require to sample over completely independently generated large networks that individually properly sample all possible boolean functions.This is not computationally feasible for K>4.Nevertheless it is feasible to perform reasonably unbiased sample of possible boolean functions for K=5that may be representative of the large scale bulk network behaviour.The results of this are shown infigure2.At K<2the nodes tend to order,fairly rapidly, whereas for K>2the system tends to remain decorrelated to an extend dependent upon K.For higher K the tendency is for more and more nodes to remain changing.The curves shown are reasonably smooth and show convergence to definite values.This is due to the large network size, relative to the number of possible boolean functions.Figure3shows the cluster size distribution for a system generated with128,000nodes for K=1. At higher K values the system is fully connected with a single ing this algorithm at K=1however,there is a distribution of islands of nodes,clustered as shown.A log-log plot reveals that cluster size population goes as cluster size s to the power of≈−1.5.5Discussion and ConclusionsWe have shown how relatively large scale random boolean networks can be simulated for K=1,...,5 and that a suitable data structure can be managed for K=6providing some means of generating unbiased and representative samples of the possible boolean functions can be provided.One such mechanism is to store a copy of the particular K-input boolean function for each network node. An arbitrary precision arithmetic package could then be used to generate the(long)look-up tables required for a high connectivity node.This will be feasible to implement in memory providing most nodes have a relatively small connectivity,K.This then provides us with a platform for investigating scale-free networks,where the distribution of connectivities is indeed exponential in K.We have shown the memory complexity and typical performance achieved using various bit storage types in the D programming language.We believe this language has great promise for numerical simulations such as the study of random boolean networks.AcknowledgmentsThis work has benefited from the use of Massey University’s“Monte”compute cluster and thanks also to the Allan Wilson centre for Molecular Biology for the use of the“Helix”Supercomputers.Figure2:The1-step correlation fraction for an RBN for different connectivities K=1,2,3,4over 128steps.Simulating Large Random Boolean Networks43References[1]Aldana,Maximino,Boolean dynamics of networks with scale-free topology,Physica D185(2003)45-66.[2]The D Programming Language,/wiki4d/wiki.cgi?-LanguageSpecification,accessed May2007.[3]Derrida,B.,and Pomeau,Y.,Random Networks of Automata:A simple annealed approxi-mation,Europhys.Lett.1(2)45-49,1986.[4]Digital Mars,D Compiler,/d/,accessed May2007.[5]Gershenson,Carlos,Introduction to Random Boolean Networks,arXiv:nlin/0408006v312August,2004.[6]Gershenson,Carlos,RBNLab,http://homepages.vub.ac.be/˜cgershen/rbn/,accessed May2007.[7]GNU D Compiler,/,accessed May2007.[8]Harvey,I.and Bossomaier,T.,Time out of joint:Attractors in Asynchronous Random BooleanNetworks,In Proc Fourth European Conference on Artificial Life(ECAL97),pp67-75,MIT Press,ed.P.Husbands and Harvey,I.,1997.[9]Kadanoff,Leo,Coppersmith,Susan and Aldana,Maximino,Boolean Dynamics with RandomCouplings,arXiv:nlin/0204062v2,2002.[10]Kauffman,S.A.,Metabolic stability and epigenesis in randomly constructed genetic nets,Journal of Theoretical Biology,22:437–467,1969.[11]Kauffman,S.A.,The Origins of Order,Oxford University Press,1993.[12]Wolfram,Stephen,Theory and Applications of Cellular Automata,Pub.World Scientific,1986.[13]Wuensche,A.,Discrete Dynamical networks and their attractor basins,In plexSystems1998pp3-21.ed R.Standish et al.,UNSW Sydney,Australia.。
斯普林格数学研究生教材丛书

《斯普林格数学研究生教材丛书》(Graduate Texts in Mathematics)GTM001《Introduction to Axiomatic Set Theory》Gaisi Takeuti, Wilson M.Zaring GTM002《Measure and Category》John C.Oxtoby(测度和范畴)(2ed.)GTM003《Topological Vector Spaces》H.H.Schaefer, M.P.Wolff(2ed.)GTM004《A Course in Homological Algebra》P.J.Hilton, U.Stammbach(2ed.)(同调代数教程)GTM005《Categories for the Working Mathematician》Saunders Mac Lane(2ed.)GTM006《Projective Planes》Daniel R.Hughes, Fred C.Piper(投射平面)GTM007《A Course in Arithmetic》Jean-Pierre Serre(数论教程)GTM008《Axiomatic set theory》Gaisi Takeuti, Wilson M.Zaring(2ed.)GTM009《Introduction to Lie Algebras and Representation Theory》James E.Humphreys(李代数和表示论导论)GTM010《A Course in Simple-Homotopy Theory》M.M CohenGTM011《Functions of One Complex VariableⅠ》John B.ConwayGTM012《Advanced Mathematical Analysis》Richard BealsGTM013《Rings and Categories of Modules》Frank W.Anderson, Kent R.Fuller(环和模的范畴)(2ed.)GTM014《Stable Mappings and Their Singularities》Martin Golubitsky, Victor Guillemin (稳定映射及其奇点)GTM015《Lectures in Functional Analysis and Operator Theory》Sterling K.Berberian GTM016《The Structure of Fields》David J.Winter(域结构)GTM017《Random Processes》Murray RosenblattGTM018《Measure Theory》Paul R.Halmos(测度论)GTM019《A Hilbert Space Problem Book》Paul R.Halmos(希尔伯特问题集)GTM020《Fibre Bundles》Dale Husemoller(纤维丛)GTM021《Linear Algebraic Groups》James E.Humphreys(线性代数群)GTM022《An Algebraic Introduction to Mathematical Logic》Donald W.Barnes, John M.MackGTM023《Linear Algebra》Werner H.Greub(线性代数)GTM024《Geometric Functional Analysis and Its Applications》Paul R.HolmesGTM025《Real and Abstract Analysis》Edwin Hewitt, Karl StrombergGTM026《Algebraic Theories》Ernest G.ManesGTM027《General Topology》John L.Kelley(一般拓扑学)GTM028《Commutative Algebra》VolumeⅠOscar Zariski, Pierre Samuel(交换代数)GTM029《Commutative Algebra》VolumeⅡOscar Zariski, Pierre Samuel(交换代数)GTM030《Lectures in Abstract AlgebraⅠ.Basic Concepts》Nathan Jacobson(抽象代数讲义Ⅰ基本概念分册)GTM031《Lectures in Abstract AlgebraⅡ.Linear Algabra》Nathan.Jacobson(抽象代数讲义Ⅱ线性代数分册)GTM032《Lectures in Abstract AlgebraⅢ.Theory of Fields and Galois Theory》Nathan.Jacobson(抽象代数讲义Ⅲ域和伽罗瓦理论)GTM033《Differential Topology》Morris W.Hirsch(微分拓扑)GTM034《Principles of Random Walk》Frank Spitzer(2ed.)(随机游动原理)GTM035《Several Complex Variables and Banach Algebras》Herbert Alexander, John Wermer(多复变和Banach代数)GTM036《Linear Topological Spaces》John L.Kelley, Isaac Namioka(线性拓扑空间)GTM037《Mathematical Logic》J.Donald Monk(数理逻辑)GTM038《Several Complex Variables》H.Grauert, K.FritzsheGTM039《An Invitation to C*-Algebras》William Arveson(C*-代数引论)GTM040《Denumerable Markov Chains》John G.Kemeny, urie Snell, Anthony W.KnappGTM041《Modular Functions and Dirichlet Series in Number Theory》Tom M.Apostol (数论中的模函数和Dirichlet序列)GTM042《Linear Representations of Finite Groups》Jean-Pierre Serre(有限群的线性表示)GTM043《Rings of Continuous Functions》Leonard Gillman, Meyer JerisonGTM044《Elementary Algebraic Geometry》Keith KendigGTM045《Probability TheoryⅠ》M.Loève(概率论Ⅰ)(4ed.)GTM046《Probability TheoryⅡ》M.Loève(概率论Ⅱ)(4ed.)GTM047《Geometric Topology in Dimensions 2 and 3》Edwin E.MoiseGTM048《General Relativity for Mathematicians》Rainer.K.Sachs, H.Wu伍鸿熙(为数学家写的广义相对论)GTM049《Linear Geometry》K.W.Gruenberg, A.J.Weir(2ed.)GTM050《Fermat's Last Theorem》Harold M.EdwardsGTM051《A Course in Differential Geometry》Wilhelm Klingenberg(微分几何教程)GTM052《Algebraic Geometry》Robin Hartshorne(代数几何)GTM053《A Course in Mathematical Logic for Mathematicians》Yu.I.Manin(2ed.)GTM054《Combinatorics with Emphasis on the Theory of Graphs》Jack E.Graver, Mark E.WatkinsGTM055《Introduction to Operator TheoryⅠ》Arlen Brown, Carl PearcyGTM056《Algebraic Topology:An Introduction》W.S.MasseyGTM057《Introduction to Knot Theory》Richard.H.Crowell, Ralph.H.FoxGTM058《p-adic Numbers, p-adic Analysis, and Zeta-Functions》Neal Koblitz(p-adic 数、p-adic分析和Z函数)GTM059《Cyclotomic Fields》Serge LangGTM060《Mathematical Methods of Classical Mechanics》V.I.Arnold(经典力学的数学方法)(2ed.)GTM061《Elements of Homotopy Theory》George W.Whitehead(同论论基础)GTM062《Fundamentals of the Theory of Groups》M.I.Kargapolov, Ju.I.Merzljakov GTM063《Modern Graph Theory》Béla BollobásGTM064《Fourier Series:A Modern Introduction》VolumeⅠ(2ed.)R.E.Edwards(傅里叶级数)GTM065《Differential Analysis on Complex Manifolds》Raymond O.Wells, Jr.(3ed.)GTM066《Introduction to Affine Group Schemes》William C.Waterhouse(仿射群概型引论)GTM067《Local Fields》Jean-Pierre Serre(局部域)GTM069《Cyclotomic FieldsⅠandⅡ》Serge LangGTM070《Singular Homology Theory》William S.MasseyGTM071《Riemann Surfaces》Herschel M.Farkas, Irwin Kra(黎曼曲面)GTM072《Classical Topology and Combinatorial Group Theory》John Stillwell(经典拓扑和组合群论)GTM073《Algebra》Thomas W.Hungerford(代数)GTM074《Multiplicative Number Theory》Harold Davenport(乘法数论)(3ed.)GTM075《Basic Theory of Algebraic Groups and Lie Algebras》G.P.HochschildGTM076《Algebraic Geometry:An Introduction to Birational Geometry of Algebraic Varieties》Shigeru IitakaGTM077《Lectures on the Theory of Algebraic Numbers》Erich HeckeGTM078《A Course in Universal Algebra》Stanley Burris, H.P.Sankappanavar(泛代数教程)GTM079《An Introduction to Ergodic Theory》Peter Walters(遍历性理论引论)GTM080《A Course in_the Theory of Groups》Derek J.S.RobinsonGTM081《Lectures on Riemann Surfaces》Otto ForsterGTM082《Differential Forms in Algebraic Topology》Raoul Bott, Loring W.Tu(代数拓扑中的微分形式)GTM083《Introduction to Cyclotomic Fields》Lawrence C.Washington(割圆域引论)GTM084《A Classical Introduction to Modern Number Theory》Kenneth Ireland, Michael Rosen(现代数论经典引论)GTM085《Fourier Series A Modern Introduction》Volume 1(2ed.)R.E.Edwards GTM086《Introduction to Coding Theory》J.H.van Lint(3ed .)GTM087《Cohomology of Groups》Kenneth S.Brown(上同调群)GTM088《Associative Algebras》Richard S.PierceGTM089《Introduction to Algebraic and Abelian Functions》Serge Lang(代数和交换函数引论)GTM090《An Introduction to Convex Polytopes》Ame BrondstedGTM091《The Geometry of Discrete Groups》Alan F.BeardonGTM092《Sequences and Series in BanachSpaces》Joseph DiestelGTM093《Modern Geometry-Methods and Applications》(PartⅠ.The of geometry Surfaces Transformation Groups and Fields)B.A.Dubrovin, A.T.Fomenko, S.P.Novikov (现代几何学方法和应用)GTM094《Foundations of Differentiable Manifolds and Lie Groups》Frank W.Warner(可微流形和李群基础)GTM095《Probability》A.N.Shiryaev(2ed.)GTM096《A Course in Functional Analysis》John B.Conway(泛函分析教程)GTM097《Introduction to Elliptic Curves and Modular Forms》Neal Koblitz(椭圆曲线和模形式引论)GTM098《Representations of Compact Lie Groups》Theodor Breöcker, Tammo tom DieckGTM099《Finite Reflection Groups》L.C.Grove, C.T.Benson(2ed.)GTM100《Harmonic Analysis on Semigroups》Christensen Berg, Jens Peter Reus Christensen, Paul ResselGTM101《Galois Theory》Harold M.Edwards(伽罗瓦理论)GTM102《Lie Groups, Lie Algebras, and Their Representation》V.S.Varadarajan(李群、李代数及其表示)GTM103《Complex Analysis》Serge LangGTM104《Modern Geometry-Methods and Applications》(PartⅡ.Geometry and Topology of Manifolds)B.A.Dubrovin, A.T.Fomenko, S.P.Novikov(现代几何学方法和应用)GTM105《SL₂ (R)》Serge Lang(SL₂ (R)群)GTM106《The Arithmetic of Elliptic Curves》Joseph H.Silverman(椭圆曲线的算术理论)GTM107《Applications of Lie Groups to Differential Equations》Peter J.Olver(李群在微分方程中的应用)GTM108《Holomorphic Functions and Integral Representations in Several Complex Variables》R.Michael RangeGTM109《Univalent Functions and Teichmueller Spaces》Lehto OlliGTM110《Algebraic Number Theory》Serge Lang(代数数论)GTM111《Elliptic Curves》Dale Husemoeller(椭圆曲线)GTM112《Elliptic Functions》Serge Lang(椭圆函数)GTM113《Brownian Motion and Stochastic Calculus》Ioannis Karatzas, Steven E.Shreve (布朗运动和随机计算)GTM114《A Course in Number Theory and Cryptography》Neal Koblitz(数论和密码学教程)GTM115《Differential Geometry:Manifolds, Curves, and Surfaces》M.Berger, B.Gostiaux GTM116《Measure and Integral》Volume1 John L.Kelley, T.P.SrinivasanGTM117《Algebraic Groups and Class Fields》Jean-Pierre Serre(代数群和类域)GTM118《Analysis Now》Gert K.Pedersen(现代分析)GTM119《An introduction to Algebraic Topology》Jossph J.Rotman(代数拓扑导论)GTM120《Weakly Differentiable Functions》William P.Ziemer(弱可微函数)GTM121《Cyclotomic Fields》Serge LangGTM122《Theory of Complex Functions》Reinhold RemmertGTM123《Numbers》H.-D.Ebbinghaus, H.Hermes, F.Hirzebruch, M.Koecher, K.Mainzer, J.Neukirch, A.Prestel, R.Remmert(2ed.)GTM124《Modern Geometry-Methods and Applications》(PartⅢ.Introduction to Homology Theory)B.A.Dubrovin, A.T.Fomenko, S.P.Novikov(现代几何学方法和应用)GTM125《Complex Variables:An introduction》Garlos A.Berenstein, Roger Gay GTM126《Linear Algebraic Groups》Armand Borel(线性代数群)GTM127《A Basic Course in Algebraic Topology》William S.Massey(代数拓扑基础教程)GTM128《Partial Differential Equations》Jeffrey RauchGTM129《Representation Theory:A First Course》William Fulton, Joe HarrisGTM130《Tensor Geometry》C.T.J.Dodson, T.Poston(张量几何)GTM131《A First Course in Noncommutative Rings》m(非交换环初级教程)GTM132《Iteration of Rational Functions:Complex Analytic Dynamical Systems》AlanF.Beardon(有理函数的迭代:复解析动力系统)GTM133《Algebraic Geometry:A First Course》Joe Harris(代数几何)GTM134《Coding and Information Theory》Steven RomanGTM135《Advanced Linear Algebra》Steven RomanGTM136《Algebra:An Approach via Module Theory》William A.Adkins, Steven H.WeintraubGTM137《Harmonic Function Theory》Sheldon Axler, Paul Bourdon, Wade Ramey(调和函数理论)GTM138《A Course in Computational Algebraic Number Theory》Henri Cohen(计算代数数论教程)GTM139《Topology and Geometry》Glen E.BredonGTM140《Optima and Equilibria:An Introduction to Nonlinear Analysis》Jean-Pierre AubinGTM141《A Computational Approach to Commutative Algebra》Gröbner Bases, Thomas Becker, Volker Weispfenning, Heinz KredelGTM142《Real and Functional Analysis》Serge Lang(3ed.)GTM143《Measure Theory》J.L.DoobGTM144《Noncommutative Algebra》Benson Farb, R.Keith DennisGTM145《Homology Theory:An Introduction to Algebraic Topology》James W.Vick(同调论:代数拓扑简介)GTM146《Computability:A Mathematical Sketchbook》Douglas S.BridgesGTM147《Algebraic K-Theory and Its Applications》Jonathan Rosenberg(代数K理论及其应用)GTM148《An Introduction to the Theory of Groups》Joseph J.Rotman(群论入门)GTM149《Foundations of Hyperbolic Manifolds》John G.Ratcliffe(双曲流形基础)GTM150《Commutative Algebra with a view toward Algebraic Geometry》David EisenbudGTM151《Advanced Topics in the Arithmetic of Elliptic Curves》Joseph H.Silverman(椭圆曲线的算术高级选题)GTM152《Lectures on Polytopes》Günter M.ZieglerGTM153《Algebraic Topology:A First Course》William Fulton(代数拓扑)GTM154《An introduction to Analysis》Arlen Brown, Carl PearcyGTM155《Quantum Groups》Christian Kassel(量子群)GTM156《Classical Descriptive Set Theory》Alexander S.KechrisGTM157《Integration and Probability》Paul MalliavinGTM158《Field theory》Steven Roman(2ed.)GTM159《Functions of One Complex Variable VolⅡ》John B.ConwayGTM160《Differential and Riemannian Manifolds》Serge Lang(微分流形和黎曼流形)GTM161《Polynomials and Polynomial Inequalities》Peter Borwein, Tamás Erdélyi(多项式和多项式不等式)GTM162《Groups and Representations》J.L.Alperin, Rowen B.Bell(群及其表示)GTM163《Permutation Groups》John D.Dixon, Brian Mortime rGTM164《Additive Number Theory:The Classical Bases》Melvyn B.NathansonGTM165《Additive Number Theory:Inverse Problems and the Geometry of Sumsets》Melvyn B.NathansonGTM166《Differential Geometry:Cartan's Generalization of Klein's Erlangen Program》R.W.SharpeGTM167《Field and Galois Theory》Patrick MorandiGTM168《Combinatorial Convexity and Algebraic Geometry》Günter Ewald(组合凸面体和代数几何)GTM169《Matrix Analysis》Rajendra BhatiaGTM170《Sheaf Theory》Glen E.Bredon(2ed.)GTM171《Riemannian Geometry》Peter Petersen(黎曼几何)GTM172《Classical Topics in Complex Function Theory》Reinhold RemmertGTM173《Graph Theory》Reinhard Diestel(图论)(3ed.)GTM174《Foundations of Real and Abstract Analysis》Douglas S.Bridges(实分析和抽象分析基础)GTM175《An Introduction to Knot Theory》W.B.Raymond LickorishGTM176《Riemannian Manifolds:An Introduction to Curvature》John M.LeeGTM177《Analytic Number Theory》Donald J.Newman(解析数论)GTM178《Nonsmooth Analysis and Control Theory》F.H.clarke, Yu.S.Ledyaev, R.J.Stern, P.R.Wolenski(非光滑分析和控制论)GTM179《Banach Algebra Techniques in Operator Theory》Ronald G.Douglas(2ed.)GTM180《A Course on Borel Sets》S.M.Srivastava(Borel 集教程)GTM181《Numerical Analysis》Rainer KressGTM182《Ordinary Differential Equations》Wolfgang WalterGTM183《An introduction to Banach Spaces》Robert E.MegginsonGTM184《Modern Graph Theory》Béla Bollobás(现代图论)GTM185《Using Algebraic Geomety》David A.Cox, John Little, Donal O’Shea(应用代数几何)GTM186《Fourier Analysis on Number Fields》Dinakar Ramakrishnan, Robert J.Valenza GTM187《Moduli of Curves》Joe Harris, Ian Morrison(曲线模)GTM188《Lectures on the Hyperreals:An Introduction to Nonstandard Analysis》Robert GoldblattGTM189《Lectures on Modules and Rings》m(模和环讲义)GTM190《Problems in Algebraic Number Theory》M.Ram Murty, Jody Esmonde(代数数论中的问题)GTM191《Fundamentals of Differential Geometry》Serge Lang(微分几何基础)GTM192《Elements of Functional Analysis》Francis Hirsch, Gilles LacombeGTM193《Advanced Topics in Computational Number Theory》Henri CohenGTM194《One-Parameter Semigroups for Linear Evolution Equations》Klaus-Jochen Engel, Rainer Nagel(线性发展方程的单参数半群)GTM195《Elementary Methods in Number Theory》Melvyn B.Nathanson(数论中的基本方法)GTM196《Basic Homological Algebra》M.Scott OsborneGTM197《The Geometry of Schemes》David Eisenbud, Joe HarrisGTM198《A Course in p-adic Analysis》Alain M.RobertGTM199《Theory of Bergman Spaces》Hakan Hedenmalm, Boris Korenblum, Kehe Zhu(Bergman空间理论)GTM200《An Introduction to Riemann-Finsler Geometry》D.Bao, S.-S.Chern, Z.Shen GTM201《Diophantine Geometry An Introduction》Marc Hindry, Joseph H.Silverman GTM202《Introduction to Topological Manifolds》John M.LeeGTM203《The Symmetric Group》Bruce E.SaganGTM204《Galois Theory》Jean-Pierre EscofierGTM205《Rational Homotopy Theory》Yves Félix, Stephen Halperin, Jean-Claude Thomas(有理同伦论)GTM206《Problems in Analytic Number Theory》M.Ram MurtyGTM207《Algebraic Graph Theory》Chris Godsil, Gordon Royle(代数图论)GTM208《Analysis for Applied Mathematics》Ward CheneyGTM209《A Short Course on Spectral Theory》William Arveson(谱理论简明教程)GTM210《Number Theory in Function Fields》Michael RosenGTM211《Algebra》Serge Lang(代数)GTM212《Lectures on Discrete Geometry》Jiri Matousek(离散几何讲义)GTM213《From Holomorphic Functions to Complex Manifolds》Klaus Fritzsche, Hans Grauert(从正则函数到复流形)GTM214《Partial Differential Equations》Jüergen Jost(偏微分方程)GTM215《Algebraic Functions and Projective Curves》David M.Goldschmidt(代数函数和投影曲线)GTM216《Matrices:Theory and Applications》Denis Serre(矩阵:理论及应用)GTM217《Model Theory An Introduction》David Marker(模型论引论)GTM218《Introduction to Smooth Manifolds》John M.Lee(光滑流形引论)GTM219《The Arithmetic of Hyperbolic 3-Manifolds》Colin Maclachlan, Alan W.Reid GTM220《Smooth Manifolds and Observables》Jet Nestruev(光滑流形和直观)GTM221《Convex Polytopes》Branko GrüenbaumGTM222《Lie Groups, Lie Algebras, and Representations》Brian C.Hall(李群、李代数和表示)GTM223《Fourier Analysis and its Applications》Anders Vretblad(傅立叶分析及其应用)GTM224《Metric Structures in Differential Geometry》Gerard Walschap(微分几何中的度量结构)GTM225《Lie Groups》Daniel Bump(李群)GTM226《Spaces of Holomorphic Functions in the Unit Ball》Kehe Zhu(单位球内的全纯函数空间)GTM227《Combinatorial Commutative Algebra》Ezra Miller, Bernd Sturmfels(组合交换代数)GTM228《A First Course in Modular Forms》Fred Diamond, Jerry Shurman(模形式初级教程)GTM229《The Geometry of Syzygies》David Eisenbud(合冲几何)GTM230《An Introduction to Markov Processes》Daniel W.Stroock(马尔可夫过程引论)GTM231《Combinatorics of Coxeter Groups》Anders Bjröner, Francesco Brenti(Coxeter 群的组合学)GTM232《An Introduction to Number Theory》Graham Everest, Thomas Ward(数论入门)GTM233《Topics in Banach Space Theory》Fenando Albiac, Nigel J.Kalton(Banach空间理论选题)GTM234《Analysis and Probability:Wavelets, Signals, Fractals》Palle E.T.Jorgensen(分析与概率)GTM235《Compact Lie Groups》Mark R.Sepanski(紧致李群)GTM236《Bounded Analytic Functions》John B.Garnett(有界解析函数)GTM237《An Introduction to Operators on the Hardy-Hilbert Space》Rubén A.Martínez-Avendano, Peter Rosenthal(哈代-希尔伯特空间算子引论)GTM238《A Course in Enumeration》Martin Aigner(枚举教程)GTM239《Number Theory:VolumeⅠTools and Diophantine Equations》Henri Cohen GTM240《Number Theory:VolumeⅡAnalytic and Modern Tools》Henri Cohen GTM241《The Arithmetic of Dynamical Systems》Joseph H.SilvermanGTM242《Abstract Algebra》Pierre Antoine Grillet(抽象代数)GTM243《Topological Methods in Group Theory》Ross GeogheganGTM244《Graph Theory》J.A.Bondy, U.S.R.MurtyGTM245《Complex Analysis:In the Spirit of Lipman Bers》Jane P.Gilman, Irwin Kra, Rubi E.RodriguezGTM246《A Course in Commutative Banach Algebras》Eberhard KaniuthGTM247《Braid Groups》Christian Kassel, Vladimir TuraevGTM248《Buildings Theory and Applications》Peter Abramenko, Kenneth S.Brown GTM249《Classical Fourier Analysis》Loukas Grafakos(经典傅里叶分析)GTM250《Modern Fourier Analysis》Loukas Grafakos(现代傅里叶分析)GTM251《The Finite Simple Groups》Robert A.WilsonGTM252《Distributions and Operators》Gerd GrubbGTM253《Elementary Functional Analysis》Barbara D.MacCluerGTM254《Algebraic Function Fields and Codes》Henning StichtenothGTM255《Symmetry Representations and Invariants》Roe Goodman, Nolan R.Wallach GTM256《A Course in Commutative Algebra》Kemper GregorGTM257《Deformation Theory》Robin HartshorneGTM258《Foundation of Optimization》Osman GülerGTM259《Ergodic Theory:with a view towards Number Theory》Manfred Einsiedler, Thomas WardGTM260《Monomial Ideals》Jurgen Herzog, Takayuki HibiGTM261《Probability and Stochastics》Erhan CinlarGTM262《Essentials of Integration Theory for Analysis》Daniel W.StroockGTM263《Analysis on Fock Spaces》Kehe ZhuGTM264《Functional Analysis, Calculus of Variations and Optimal Control》Francis ClarkeGTM265《Unbounded Self-adjoint Operatorson Hilbert Space》Konrad Schmüdgen GTM266《Calculus Without Derivatives》Jean-Paul PenotGTM267《Quantum Theory for Mathematicians》Brian C.HallGTM268《Geometric Analysis of the Bergman Kernel and Metric》Steven G.Krantz GTM269《Locally Convex Spaces》M.Scott Osborne。
university and their function

•
• (P3)It enables man to construct an intellectual vision of a new world , and it preserves the zest of life by the suggestion of satisfying purposes. • People with imagination will be able to form a new outlook which is different from that of people without imagination. Imagination is capable of preserving people’s enthusiasm for life because it can show people that life has many purposes which can be pleasing.
• Alfred North Whitehead (1861– 1947) was a British mathematician, logician and philosopher best known for his work in mathematical logic and the philosophy of science. In collaboration with Bertrand Russell, he authored the landmark three-volume Principia Mathematica《数学原理》 (1910, 1912, 1913) and contributed significantly to twentieth-century logic, philosophy of science and metaphysics形而上学.
雅克布逊

一:语言符号角度看翻译
受到索绪尔的启迪,雅克布逊从符号学出 发认为人们对词意的理解,并不取决于生 活经验以及对世界的认识,而是取决于人 们赋予该词的含义。 要知道一词的含义,并不需要见过或者使 用过,只要知道它被赋予的意义。(见书 上例子)
语言虽然有差异,在语法范畴中的复杂性 却并不妨碍概念之间的相互转换。
只有诗歌翻译中,诗歌的某些音素的相似 性不可以用语义来传递,这时就需要译者 的创造性转换。
雅克布逊在看待诗歌翻译上,持有不可译 观点。这是因为诗歌中双关语语音语义所 建立的关联是不可译的,只可能进行创造 性移植。
•谢谢
Brief Introduction
到20世纪中叶,翻译理论家对语言 理论更加感兴趣,著名的翻译理论 家霍姆斯,雅克布逊,卡特福德, 奈达等等都建立了以语言学为基础 的翻译翻译理论。本章注重探讨翻 译中等值翻译的相关成果。
纽马克的语义翻译(Semantic translation)和交际翻译 (Communicative translation)
奈达极具创意的形式对等 (formal equivalence)以及动态 对等(dynamic quivalence) 等效原则(The principle of
effect)
德国翻译理论学家科勒(Werner Koller)进一步发展了奈达的对等 理论,提出了内涵对等,外延对等, 文本规范对等,语用对等以及形式 对等。
On linguistic aspects of translation
1. Intralingual translation 语内翻译——某一 语言内部,为着某种目的进行的词句意义 的转换
Nonlinear Functional Analysis

Nonlinear Functional Analysis,Jerry Bona Day 1We begin with a short historical sketch of nonlinear functional analysis (NFA).During the mid to late 1800s,beginnings of these analytical techniques appeared in the works of the French Poincar´e and Boussinesq.However,the first paper properly on NFA appeared around 1926,written by G.D.Birkhoffand Kellogg.During the 1930s,this work was expanded by Schauder and Leray,followed by Levrentief in the 1940s.Finally,during the 1950s,the subject really burgeoned with the work of Browder and many others.Classical Fixed Point TheoremsThe two primary theorems we shall introduce today are the Schauder fixed point theorem and the Picard-Banach fixed point theorem,often known in a slightly different form as the Contraction Mapping Principle.Vocabulary:Let X be a topological space and U ={U α}α∈I be a collection of open subsets of X such that αU α=X .Then,X is paracompact if every open cover of X has a locally finite subcover;that is,for any x ∈X there exists a neighborhood V containing x such that V U β=∅except for a finite number of U β.A further result is that any paracompact space has a partition of unity .That is,let X be paracompact,with open cover {U α}.Then there exist continuous functions φα:U α→[0,1]having supp(φα)⊂U αsuch that for every x ∈X , α I φα(x )=1where φα(x )=0except for a finite number of α.Prove this result by applying Urysohn’s Lemma.The next theorem is a generalization of Brouwer’s fixed point theorem in finite dimen-sions.Theorem (Schauder):Let C be a compact,convex subset of a Banach space X .let f :C →C be continuous.Then,f has a fixed point.Proof:(not Schauder’s original approach)Let ε>0.Write C = x ∈C B ε(x ).By com-pactness,take a finite subcover:C ⊂ N i =1B ε(x i ).By paracompactness,we may constructa partition of unity associated to this cover:that is,we have nonnegative {φi }N i =1such that N i =1φi (x )=1∀x ∈C and supp(φi )⊂B ε(x i )for 1≤i ≤N .Let f ε:C →C be given by the convex combination of x i ,f ε(x )= Ni =1φi (f (x ))x i .Notice that f εis a finite sum of continuous maps and,thus,is itself continuous.By convexity of C ,we have f ε(x )∈C .In fact,f ε:C →C ε=Co (x 1,...,x N )⊂C ,where C εdenotes the convex hull.Now,for x ∈C ,we compute|f (x )−f ε(x )|= N i =1φi (f (x ))(f (x )−x i ) ≤Ni =1φi (f (x ))ε=ε,since f (x )∈B ε(x i )for some i (as the B εsets form an open cover of C .Thus,we have the fact that sup x ∈C |f (x )−f ε(x )|≤ε.Now consider f ε|C ε:C ε→C ε.By Brouwer’s theorem,we know that for each εthere exists a fixed point x ε∈C εsuch that f ε(x ε)=x ε.Notice that we have a net {x ε}ε>0.Since C is compact,there must exist a subsequence of indices {εn }∞n =1⊂R +so that εn 0;hence,x εn must converge to some x ∞.Since f is continuous,it follows that f (x εn )→f (x ∞).However,f (x εn )=f (x εn )−x εn +x εn =f (x εn )−f εn (x εn )+x εn →0+x ∞.One might be concerned about uniform convergence in this context.If so,use a norm argument: f (x εn )−x ∞ ≤ f (x εn )−f εn (x εn ) + f εn (x εn )−x ∞≤small + x εn −x ∞but x εn −x ∞ →0.Thus,f (x ∞)=x ∞,which shows that f has a fixed point.We need a little more notation in order to state the next theorem,familiar from previous studies.Vocabulary:For x 1∈M ,a metric space,the orbit of x 1under the map f :M →M isO f (x 1)={x 1,f (x 1),f (f (x 1)),f 3(x 1),...,f n (x 1),...}.Theorem (Contraction Mapping Principle):Let M be a complete metric space,with metric d .Let f :M →M be a contraction,i.e.,d (f (x ),f (y ))≤θd (x,y )for a fixed θ<1.Then,there exists a unique x 0∈M such that f (x 0)=x 0and furthermore,for any x 1∈M ,f n (x 1)→x 0.Theorem :Let M be a complete,bounded metric space.Suppose there exists a continuous φ:R +→R +such that φ(0)=0and φ(r )<r for all r >0.Let f :M →M be such that d (f (x ),f (y ))≤φ(d (x,y )).Then,f has a unique fixed point x ∞∈M ,such that x ∞=lim n →∞f n x 0for any x 0∈M .Notice that without loss of generality,we may assume thatφis increasing.This is due to the fact that we may makeφmonotone for free by defining˜φ(r)=max0≤s≤rφ(s). Proof:Uniqueness.Suppose x and¯x arefixed points with x=¯x.Then,0<d(x,¯x)=d(f(x),f(¯x))≤φ(d(x,¯x))<d(x,¯x),a contradiction unless x=¯x.Existence,by contradiction.Let x0∈M.Letd(f i x0,f k x0).d j=diam{O f(f j x0)}=supi,k≥jWe know that d j<∞since M is bounded.Suppose that d j→0.Then,O f(x0)is a Cauchy sequence,which perforce converges:f j(x0)→x∞as j→∞.Thus,f j+1(x0)→x∞but by continuity of f,f j+1(x0)=f(f j(x0))→f(x∞).Now,d j+1=supd(f r+1(x0),f s+1(x0))r,x≥jd(f(f r(x0)),f(f s(x0)))=supr,s≥jφ(d(f r(x0),f s(x0)))≤supr,s≥j≤supd(f r(x0),f s(x0))=d jr,s≥jwhich implies that{d j}∞j=1is a decreasing sequence of real,nonnegative numbers.Hence, there must exist a limit,d∞=lim j→∞d j.We want to show that d∞=0.Suppose not.Then,by continuity of the functionφ,φ(d j)→φ(d∞).However,by monotonicity,φ(d j)≥d j+1→d∞,which forcesφ(d∞)≥d∞.This contradicts the condition that φ(d∞)<d∞,unless d∞=0.Therefore,d∞=0.Problem:Use this result to prove the Contraction Mapping Principle stated earlier.The difficulty lies in the fact that the CMP does not require boundedness.Theorem(Variant of the above):Make the same assumptions about M,φand f except that we required(f N(x),f N(y))≤φ(d(x,y)).Then,f has afixed point in M.Proof:exercise.Question:is thefixed point necessarily unique?。
Chapter11 Structure and function of ribozymes@生物化学精品讲义

1. Small ribozymes 2. Large ribozymes
Mechanism of Ribozyme Catalysis
1. Mechanism of small ribozyme catalysis 2. Mechanism of large ribozyme catalysis
Chapter 11 Structure and Function of Ribozymes
Outline
Definition and Discovery of Ribozymes Comparison between Protein-based and RNA-
based Enzymes Types of ribozymes
Types of Ribozymes
2. The range of chemical reactions that are catalyzed by ribozymes is rather limited, and the rate enhancements are relatively modest because the range of functional groups that can be called upon to participate in the chemistry is limited compared to the set of amino acid side-chains.
Crank–Nicolsonmethod
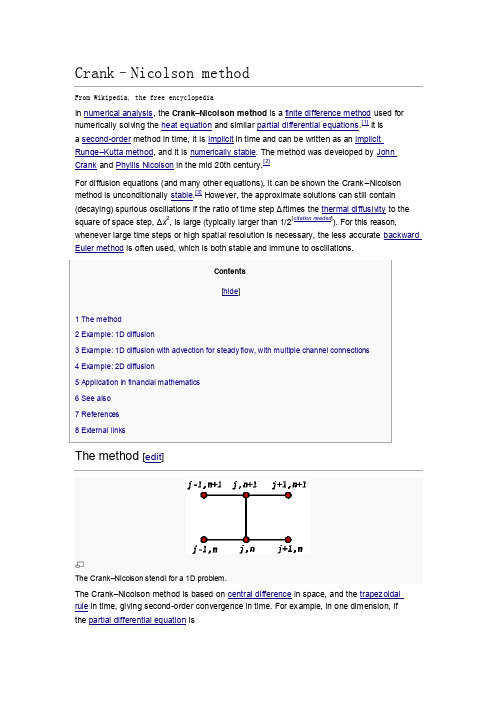
Crank–Nicolson method From Wikipedia, the free encyclopediathen, letting , the equation for Crank–Nicolson method is a combination of the forward Euler method at and the backward Euler method at n + 1 (note, however, that the method itself is not simply the average of those two methods, as the equation has an implicit dependence on the solution):The function F must be discretized spatially with a central difference.Note that this is an implicit method: to get the "next" value of u in time, asystem of algebraic equations must be solved. If the partial differentialequation is nonlinear, the discretization will also be nonlinear so thatadvancing in time will involve the solution of a system of nonlinear algebraicequations, though linearizations are possible. In many problems, especiallylinear diffusion, the algebraic problem is tridiagonal and may be efficientlysolved with the tridiagonal matrix algorithm, which gives a fast directsolution as opposed to the usual for a full matrix.The Crank–Nicolson method is often applied to diffusion problems. As anexample, for linear diffusion,whose Crank–Nicolson discretization is then:or, letting :which is a tridiagonal problem, so that may be efficientlysolved by using the tridiagonal matrix algorithm in favor of amuch more costly matrix inversion.A quasilinear equation, such as (this is a minimalistic exampleand not general)would lead to a nonlinear system of algebraic equationswhich could not be easily solved as above; however, it ispossible in some cases to linearize the problem by usingthe old value for , that is instead of .Other times, it may be possible toestimate using an explicit method and maintainstability.The Crank–Nicolson method is often applied to diffusion problems. As an example, for linear diffusion,whose Crank–Nicolson discretization is then:or, letting :which is a tridiagonal problem, so that may be efficiently solved by using the tridiagonal matrix algorithm in favor of a much more costly matrix inversion.A quasilinear equation, such as (this is a minimalistic example and not general)would lead to a nonlinear system of algebraic equations which could not beeasily solved as above; however, it is possible in some cases to linearize theproblem by using the old value for , that is instead of .Other times, it may be possible to estimate using an explicitmethod and maintain stability.。
A general statement of the functional equation for the Riemann zeta-function

a rX iv:mat h /27237v1[mat h.NT]25J u l22A GENERAL STATEMENT OF THE FUNCTIONAL EQUATION FOR THE RIEMANN ZETA-FUNCTION LUISB ´AEZ-DUARTE 1.Introduction This is a completely formal exposition.All lemmas and theorems are valid for sufficiently large,dense subspaces of H :=L 2((0,∞),dx ).We borrow in toto the notations from my paper on invariant unitary operators [1].We denote the Mellin transform of f by f ∧(s ):= ∞0x s −1f (x )dx,which is at least defined on the critical line as a Cauchy principal value in the H -sense.Following Burnol [3]denote the M¨u ntz-modified Poisson summation op-erator by P ,which acts formally on the set of all complex functions f on (0,∞)according to P f (x ):=∞ n =1f (nx )−12Γ(s ).2LUIS B´AEZ-DUARTE2.General statements of the functional equationWe recall Theorem2.1of[1]:Theorem2.1.For any skew root T,and any real-valued generator g there is a unique,bounded,invariant operator W=W(T,g)with W g=T g.W is unitary and satisfies(T W)2=(W T)2=I,W−1=T W T.Why call the following triviality a“theorem”is a question left to the reader.Do note that the operator W depends explicitly on f.Theorem2.2(Main identity).Let f be good and suppose that P f is a real valued generator.Let T be a skew root and W=W(T,P f).ThenT P f=P W f.e Theorem2.1and the commutativity Lemma1.1.We specialize the main identity to give two general statements of the functional equation of the Riemann zeta function.Theorem2.3(Functional equation I).Let f be good and suppose that P f is a real valued generator.Let W=W(S,P f)and h=W f.Thenζ(1−s)f∧(1−s)=ζ(s)h∧(s).(2.1)Proof.The main identity is now SP f=P h,where we apply the Mellin transform using Lemmas1.2,1.3.Theorem2.4(Functional equation II).Let f be good and suppose that P f is a real valued generator.Let W=W(C,P f)and h=W f.Thenζ(1−s)φ(s)f∧(1−s)=ζ(s)h∧(s).(2.2)Proof.The main identity is now C P f=P h,where we apply the Mellin transform using Lemmas1.2,1.4.For an application of Theorem2.3choose f=χ,then P f(x)=−ρ(1/x) and W=U,so thath(x)=sin(2πx)s ,h∧(s)=−21−sπ−s cosπs1−s =−21−sπs cosπsZETA FUNCTIONAL EQUATION3 I don’t yet have a genuine application of Theorem2.4.However let as usual M(x):= n≤xµ(n),and choosef(x):=M(1/x).I have shown in[2]that this f∈H;on the other hand note that P f=χ. So again W=W(C,P f)=W(C,χ)=U.If one blithely insists on applying the theorem one obtains an intriguing equation from(2.2).Remark2.1.Quite frankly I am chagrined that I have not been included other genuine examples.More important perhaps is this question:how does this relate to Burnol’s view of the functional equation in[3]?References1.Luis B´a ez-Duarte,A class of invariant unitary operators,Advances in Mathematics,144,No.1(1999),1-12.2.Luis B´a ez-Duarte,Arithmetical versions of the Nyman-Beurling criterion for the Rie-mann hypothesis,Preprint2001,to appear as New versions of the Nyman-Beurling criterion for the Riemann Hypothesis to appear in IJMMS2002.3.Jean-Fran¸c ois Burnol,Co-Poisson intertwining:distribution and function theoreticaspects,preprint,15May2002.Luis B´a ez-DuarteDepartamento de Matem´a ticasInstituto Venezolano de Investigaciones Cient´ıficasApartado21827,Caracas1020-AVenezuelalbaezd@。
法国数学家拉格朗日著作《解析函数论》英文名

法国数学家拉格朗日著作《解析函数论》英文名全文共3篇示例,供读者参考篇1Title: French Mathematician Lagrange's Work "Analytical Function Theory"Introduction:Lagrange's "Analytical Function Theory" is a seminal work by the French mathematician Joseph-Louis Lagrange, also known as the Lagrange interpolation or Lagrange polynomial. In this work, Lagrange presents a detailed analysis of functions and their properties, laying the foundation for modern function theory. The book delves into topics such as series, limits, derivatives, and integrals of functions, providing a comprehensive study of mathematical functions.Chapter 1: Historical BackgroundJoseph-Louis Lagrange was born in Turin, Italy, in 1736 and later moved to Paris, where he made significant contributions to mathematics, mechanics, and astronomy. Lagrange's work in function theory was influenced by earlier mathematicians such as Euler, d'Alembert, and Legendre. His innovative approach toanalyzing functions set him apart as a pioneering figure in the field of mathematics.Chapter 2: Analytical Function TheoryIn "Analytical Function Theory," Lagrange explores the properties of functions through the use of calculus and algebraic techniques. He introduces the concept of series as a way to represent functions as infinite sums of terms, allowing for a more precise analysis of their behavior. Lagrange also discusses the importance of limits in determining the behavior of functions at particular points, laying the groundwork for modern calculus.Chapter 3: Applications and ImpactLagrange's work on function theory has had a lasting impact on mathematics, with his ideas forming the basis for modern theories in analysis and calculus. The concept of the Lagrange interpolation polynomial, named in his honor, is still widely used in numerical analysis and approximation techniques. His work has inspired generations of mathematicians to further explore the depths of function theory and its applications in various fields.Conclusion:In conclusion, Joseph-Louis Lagrange's work "Analytical Function Theory" stands as a testament to his brilliance and innovative contributions to the field of mathematics. Through his meticulous analysis of functions and their properties, Lagrange paved the way for future developments in function theory and calculus. His work remains a cornerstone of modern mathematics, continuing to inspire mathematicians worldwide to push the boundaries of knowledge in this dynamic field.篇2Title: French Mathematician Lagrange's Work "Analytic Functions Theory"IntroductionLagrange's work on Analytic Functions Theory is a significant contribution to the field of mathematics. This book, also known as "Théorie des Fonctions Analytiques" in French, was published in the year 1797 by the renowned French mathematician Joseph Louis Lagrange. In this seminal work, Lagrange established the foundation for the study of analytic functions and laid the groundwork for the development of complex analysis.Background of LagrangeJoseph Louis Lagrange, born in Turin, Italy in 1736, was a prominent mathematician who made groundbreaking contributions to various fields of mathematics, such as number theory, calculus, and celestial mechanics. He is widely regarded as one of the greatest mathematicians of all time and his work continues to influence modern mathematics.Content of the BookIn "Analytic Functions Theory", Lagrange delves into the study of functions that can be represented by a power series expansion. He introduces key concepts and theorems related to complex analysis, such as Cauchy's integral theorem, the residue theorem, and the fundamental theorem of algebra. Lagrange's work on the properties and behavior of analytic functions revolutionized the field of mathematics and paved the way for further developments in the study of complex numbers.Significance of the WorkLagrange's book is considered a seminal work in the field of mathematics and remains a cornerstone of complex analysis. His contributions to analytic functions theory have had a lasting impact on the field of mathematics and continue to influence contemporary research in areas such as number theory, physics,and engineering. The book has been widely studied and referenced by mathematicians and scientists around the world.ConclusionIn conclusion, Lagrange's work on Analytic Functions Theory stands as a testament to his brilliance and innovation in the field of mathematics. His insights and discoveries continue to shape our understanding of complex analysis and pave the way for new advancements in the field. The book remains a timeless classic in the realm of mathematics and serves as a source of inspiration for generations of mathematicians to come.篇3Title: The Analytic Function Theory by French Mathematician LagrangeIntroduction:Joseph-Louis Lagrange, a renowned mathematician from France, made significant contributions to the field of mathematics during the 18th century. One of his most influential works is the book "Analytic Function Theory," where he laid down the foundations for the study of complex functions. In this article, we will delve into the contents of this seminal work and discuss its impact on the development of mathematics.Overview of the Book:Lagrange's "Analytic Function Theory" is a comprehensive treatise on the analysis of complex functions, which play a crucial role in a variety of mathematical disciplines including calculus, differential equations, and number theory. The book is divided into several sections, each covering different aspects of the theory of analytic functions. Lagrange begins by introducing the basic concepts of complex numbers and functions, before delving into more advanced topics such as power series, contour integration, and the Cauchy-Riemann equations.Key Concepts and Theorems:One of the key contributions of Lagrange in this work is the development of the Cauchy Integral Formula, which provides a powerful method for calculating complex integrals over closed curves. This formula has important applications in the study of harmonic functions and the theory of residues. Lagrange also proved several important theorems in the book, including the Maximum Modulus Principle and the Riemann Mapping Theorem, which have been instrumental in the development of complex analysis.Impact on Mathematics:Lagrange's "Analytic Function Theory" is considered a seminal work in the field of complex analysis and has had a lasting impact on the development of mathematics. The insights and techniques introduced by Lagrange in this book have been instrumental in solving many mathematical problems in diverse areas such as physics, engineering, and computer science. The book continues to be studied and referenced by mathematicians and researchers around the world, highlighting the enduring legacy of Lagrange's contributions to the field.Conclusion:In conclusion, Joseph-Louis Lagrange's "Analytic Function Theory" stands as a cornerstone in the field of complex analysis, providing a solid foundation for the study of analytic functions and their applications. The insights and theorems introduced by Lagrange in this book have had a profound impact on the development of mathematics, shaping the way we understand and solve complex mathematical problems. As we continue to push the boundaries of mathematical research, Lagrange's work remains as relevant and influential as ever.。
Cohesion in English

Cohesion in EnglishThe various kinds of cohesion had been out lined by MAK Halliday in his writings on stylistics and the concept was developed by Ruqayia Hasan in her University of Edinburgh doctoral thesis.Cohesive relations are relations between two or more elements in a text that are independent of the structure: for example between a personal pronoun and an antecedent proper name, such as John ….he. A semantic relation of this kind may be set up either within a sentence with the consequence that when it crosses a sentence boundary it has the effect of making the two sentences cohere with one another.The major function of cohesion is text formation. As defined: text is a unified whole of linguistic items, this unity of text as a semantic whole is source for the concept of cohesion.So first we will explore the concept of text.TextText in linguistics refers to any passage spoken written of whatever length that forms a unified whole. A reader can easily identify whether the passage he is reading is a text or otherwise a collection of unrelated sentences. A text may be: spoken, written, prose, verse, dialogue, monologue, single proverb, a single cry for help or all day discussion on a committee. A text is a unit of language in use. A grammatical unit that is larger than a sentence. A text is not something that is like a sentence only bigger or larger. It is misleading. Rather text can be best defined as a semantic unit; a unit not of form but of meaning. If it is semantic unit, we will not expect to find it in structure of a sentence as a grammatical unit as phrase, clause etc.So text is not consist of sentence but it is realized by sentence or encoded in sentences.There are certain objective factors involved that constitute a text.Constituents of Text1.Texture:2.Ties:3.Cohesion1. Texture:Texture is that feature of text which made it a unified whole.According to ‘The Concise Oxford Dictionary of Linguistics by P.H. Mathews’ cohesion and coherence are sources which create texture. Crys tal adds ‘informativeness’ to cohesion and coherence.Example:Wash and core six cooking apples. Put them into a fireproof dish.Here them reference back to six cooking apples to create cohesion between the two sentences. Here we make a presupposition about the relationship between them and six cooking apples but it is not enough only to make a presupposition rather that presupposition must be satisfied to create texture as shown in the example . These two items are co referential and this coreferentiality creates texture.Here are five cohesive devices to create texture:(i)Reference(ii)Substitution(iii)Ellipses(iv)Conjunction(v)Lexical Cohesion2. TiesThe term refers to a single instance of cohesion.Example:Wash and core six cooking apples. Put them into a fireproof dish.Them and six cooking apples show reference as tie.If we take the Example:Wash and core six cooking apples. Put the apples into a fireproof dish.Here are two ties(i)Reference(ii)RepetitionCohesive analysis of text is made in terms of tie for a systematic account of its patterns which area source for texture. Onward we will use the term ‘cohesive tie’ in place of ‘tie’.Here are five different kinds of cohesive ties that are also called cohesive devices:(i)Reference(ii)Substitution(iii)Ellipses(iv)Conjunction(v)Lexical cohesionMAKH and RH have based their model of cohesion on these cohesive ties. A detailed review is given here in the proceeding discussion.3. Cohesion‘The Concise Oxford Dictionary of Lin guisti cs by P.H. Mathews (1997)’ defines cohesion in term of syntactic unit (sentence).‘ A Dictionary Of Linguistics And Phonetics by David Crystal (1997)’ defines cohesion in terms of a grammatical unit (words)MAKH and RH (1976) argued that the concept of cohesion is semantic one. For them it refers to relation of meaning that:exists with in textgives the text texturedefines the text as textThis relation of meaning between the elements gives the reader presupposition. This is another way of approaching the notion of cohesion that presupposing and the presupposed give us a presupposition at semantic level as a relation of meaning: The one element presupposes the otheri.e. the one element cannot be decoded without the presupposed.Example:Time fliesYou can’t; they fly too quickly.You can’t (Ellipses)They (Reference)Fly (Lexical Cohesion)Types OF CohesionLanguage is multiple coding system comprising three levels of coding:➢Meaning The semantic system➢Wording The lexicogrammatical system(grammar an vocabulary)➢Sounding/writing The phonological and orthographical systemCohesive relation fit into the overall pattern of language. Cohesion is expressed partly through vocabulary and partly through grammar.:1. Grammatical Cohesion(i) Reference(ii) Substitution(iii) Ellipses2. Lexical CohesionThe distinction between grammatical cohesion and Lexical cohesion is a matter of degree and MAHK RH suggested not to go in the depth of these overlapping areas and that conjunction is on the border line of the two types mainly it is grammatical but with the lexical component so we cannot clearly distinguish between the two types.Cohesion and the Linguistics StructureTexture and StructureStructure is one mean of expressing texture. Text consists of one sentence are fairly rare but they can be single sentences as well for ExampleNo smokingWonder never ceaseBut most of the text extends beyond the confines of single sentences so structure is important in a text as structural units such as phrase, clause and sentence which express the unity of text. But our use of term Cohesion refers especially to the non structural text forming relation. They are semantic relations and the text is a semantic unit.Cohesion With in the TextSince cohesive relation is not concerned with structure, they may be found just as well with in the sentence as between sentences cohesive relation are beyond the sentences boundaries. Cohesion is semantic relation between one element in the text and some other element that is crucial for its interpretation. This other element must also be found with in the text. Cohesion refers to the range of possibilities that exist for linking something with what has gone before. The Place of Cohesion in the Linguistics SystemHalliday (1985) has described three major functional semantic components:(i)The Ideational(ii)The Interpersonal(iii)The TextualTable: the place of cohesion in the description of English functional components of semantic systemConclusionCohesion is a part of text forming component in the linguistics system. It links together the elements that are structurally unrelated through the dependence of one on the other for its interpretation. Without cohesion the semantic system cannot be effectively activated at all. Cohesive Devices(i)Reference(ii)Substitution(iii)Ellipses(iv)Conjunction(v)Lexical cohesion1. ReferenceThere are certain items in any language which cannot be interpreted semantically in their own right rather they make reference to something else within the text for their interpretation. Here is an example of referenceDoctor Foster went to Gloucester in a shower of rainHe stepped in puddle right up to his middleAnd never went there againHere in the above exampleHe refers back to Doctor FosterThere refers back to GloucesterHe and there show that information about them is retrieved elsewhere within the text. It characterizes a particular type of cohesion which is called reference. The relationship of referenceis on semantic level. The reference items must not match the grammatical item it refers to. What must match or the semantic properties of reference item in relation to the items it refers to. Reference can be sub-categorize as followReferenceExophora EndophoraAnaphora CataphoraExophoraIt indicates situational references. Anaphora signals that reference must be made to the context of situation. It is outside the text so it is called anaphoric reference.Example;Fo r he’s a jolly good fellow and so say all of us.Here text is not indicating who he is?He can be recognized by the situation in which expression is used. They are not source of cohesion because there presupposition cannot be resolved within the text rather the presupposition is found outside the text.EndophoraIt is a general name for reference within the text. This reference can be of two types.(i)Anaphora: Reference back(ii)Cataphora: Reference forwardExample:Child: Why does that one come out?Parent: That whatChild: That one.Parent: That one what?Child: That lever there that you push to let the water out.That one that lever (cataphoric reference)That lever that one (anaphoric reference)Types of referenceThere are three types of reference(i)Personal Reference(ii)Demonstrative Reference(iii)Comparative ReferenceIt is better first explain the structure of nominal group then proceed towards three types of Reference. It is because we will analyze nominal group for cohesive analysis of these cohesive devices.Nominal GroupThe logical structure of the nominal group (noun phrase) is that it consists of head with optional modifier the modifying elements include some which precede the head and some which follow it. They can be referred as Pre modifier and Post modifier respectively.ExampleThe two high stone wall along the roadside.Wall ------------ HeadThe two high stone ------------ Pre modifierAlong the roadside ------------ Post modifierThe modifier can be further subcategorized as:(i)Deictic(ii)Numerative(iii)Epithet(iv)Classifier(v)Qualifier(vi)ThingExampleTheir famous old red wine.Deictic Deictic epithet classifier thingDeterminer adjective adjective adjective nounI. Personal ReferenceIt is a reference by means of function into a speech situation through the category of the person in the form of personal pronouns. The category of persons includes the three classes of personal pronouns. The category of person includes the three classes of personal pronouns. During the communication process the speech roles are assigned to the participants through the person system as:SpeakerAddresseeIt/one are used as a generalized form for other itemsExampleIf the buyer wants to look the condition of the property, he has to have another survey. One carried out on his own behalf.Here in the above example the use of personal pronouns is a source of personal referenceBuyer he hisSurvey oneIf possessive pronouns are used, they give two more notions other than Speaker and Addressee. They are that of Possessor and Possessed as I the following exampleThat new house is John’s. I didn’t know it was hisPossessor JohnPossessed house shown by the use of his and ‘sThe following table shows the system of person for personal reference.Table: Personal ReferenceSemantic categoryGrammatical functionClassPerson:speaker (only)addressee (s), with/without other person(s)speaker and other person other person, male other person, female other persons, objects object; passage of text generalized personII. Demonstrative ReferenceIt is essentially a form of verbal pointing. The speaker identifies or points pout the referentby locating it on scale of proximity. The system of demonstrative pronoun is given in the following table.Table: Demonstrative ReferenceSemantic category Grammatical functionProximity: near far neutralExamplesLeave that there and come here.Where do you come from?I like the lions and I like the polar bears. These are my favorites and those are myfavorites too.III. Comparative ReferenceHere two types of comparison are given:(i)General Comparison(ii)Particular Comparison(i)General ComparisonHere things compared show likeness or unlikeness without considering any particular property. Likeness or unlikeness is referential property as something is can be like something else.ExampleIt’s the sa me cat as the one we saw yesterday.Its different cat from the one we saw yesterday(ii)Particular ComparisonHere comparison is made on the scale of quantity or quality it is a matter of degree compare things on this scale. In other words we can say it expresses the comparability between things. ExampleTake some more teaWe are demanding higher living standard.There are twice as many people there as the last time.Table: Comparative referenceClassGeneral comparison:Identitygeneral similaritydifference (i.e. non-identity or similarity)Particular comparison:2. SubstitutionSubstitution is replacement of one linguistic item by another. Ellipses is also a kind of Substitution where one linguistic item is replaced by nothing/ zero. Therefore it is an omission of an item.When we talk about replacement of one item by another, we mean replacement of one word/phrase with another word or phrase. We can say substitution is a relation on lexicogrammatical level. It is used to avoid repetition of a particular item. But while locating cohesion through substitution semantic is involved.ExampleMy axe is too blunt. I mist get a sharper one.You know John already knows. I think everybody does.Substitution is confined to text so exophoric substitution is rare. Most of the substitutions are endophoric and that of anaphoric type. But cataphoric substitution can also found in certain circumstances.Types of SubstitutionThere are three types of substitution.(i)Nominal Substitution(ii)Verbal Substitution(iii)Clausal substitution1.Nominal SubstitutionThere are three nominal substitutes.one, ones, same.The nominal substitute one/onesThe substitute one/ones always function as head of a nominal group and can substitute only for an item which is itself head a nominal group.ExampleI’ve heard some strange stories in my time. But this one was perhaps the strangest one of all.Note: The word other than a substitute can be used as(i)The personal pronoun one(ii)Cardinal numeral one(iii)Determiner oneThe nominal substitute sameSame typically accompanied by the presuppose an entire nominal group.ExampleA:I’ll have two poached eggs on toast, please.B: I’ll have the sameThe Same can have following expressions as:Say the sameDO the sameBe the same2. Verbal SubstitutionThe verbal substitute is do.This operates as head of a verbal group. Lexical verb is replaced by do and its position is on the final in the group.Example from AliceThe words did not come the same as they used to do.It can also substitute for a verb plus certain other elements in the clause.ExampleI don’t know the meaning of half those language words and what’s more, I don’t believe you do either.Note: The word Do other than as substituteLexical verb do (he is doing)General verb do (they did a dance)Pro-verb do {do(action), happen(event)} Clausal substitutionHere presupposed is not an element within the clause but an entire clause. So and Not are clausal substitutesExampleIs there going to be an earth quake? - it says soTypes of Clausal SubstitutionThere are three types of clausal substitution.➢Substitution of reported clause➢Substitution of conditional clause➢Substitution of modalized clause1. Substitution of Reported ClauseThe reported clausal that is substituted by so or not is always declarative whatever the mood of the presupposed clause is whether interrogative or imperative.ExampleHas everyone gone home? I hope not.I didn’t think so.(I hope not (that) every one has gone home)Is this mango ripe? – It seems so.The essential distinction to be made here is that between reports and facts. Reports can be substituted whereas facts can not, reason is that facts are encoded at semantic level while clausal substitute works at lexicogrammatical level only.2.Substitution of Conditional ClauseConditional clause are also substituted by so and not especially following if /assuming so / suppose so etc.Example11Everyone seems to think he’s guilty. If so, no doubt he’ll offer to resignWe should recognize the place when we come to it. Yes, but supposing not then what do we do?3.Substitution of Modalized ClauseSo and not also occur as substitute for clauses expressing modality.Example‘May I give you a slice?’ she said.‘Certainly not’ the red queen said.。
MODULATORS OF RIBOSOMAL FUNCTION AND IDENTIFICATIO

专利名称:MODULATORS OF RIBOSOMAL FUNCTION AND IDENTIFICATION THEREOF发明人:Thomas A. Steitz,Peter B. Moore,NenadBan,Poul Nissen,Jeffrey Hansen申请号:US11239699申请日:20050929公开号:US20060136146A1公开日:20060622专利内容由知识产权出版社提供摘要:The invention provides methods for producing high resolution crystals of ribosomes and ribosomal subunits as well as crystals produced by such methods. The invention also provides high resolution structures of ribosomal subunits either alone or in combination with protein synthesis inhibitors. The invention provides methods for identifying ribosome-related ligands and methods for designing ligands with specific ribosome-binding properties as well as ligands that may act as protein synthesis inhibitors. Thus, the methods and compositions of the invention may be used to produce ligands that are designed to specifically kill or inhibit the growth of any target organism.申请人:Thomas A. Steitz,Peter B. Moore,Nenad Ban,Poul Nissen,Jeffrey Hansen地址:Branford CT US,North Haven CT US,Zurich CH,Aarhus N DK,New Haven CT US 国籍:US,US,CH,DK,US更多信息请下载全文后查看。
The structure and function of ribosomes

The structure and function ofribosomesRibosomes are essential components of cells that assist in the process of protein synthesis. They are found in both prokaryotic and eukaryotic cells and play a crucial role in maintaining the proper functioning of cells. This article will discuss the structure and function of ribosomes in detail.Structure of Ribosomes:Ribosomes are composed of ribosomal RNA (rRNA) and proteins. The rRNA makes up about 60% of the ribosome's mass, while the proteins account for the remaining 40%. The size of ribosomes varies depending on the organism, but they are typically around 20-25 nm in diameter.Ribosomes are made up of two subunits – a small subunit and a large subunit. The small subunit is responsible for binding to messenger RNA (mRNA), while the larger subunit is responsible for catalyzing the formation of peptide bonds during protein synthesis.The small subunit contains a binding site for mRNA and a binding site for tRNA (transfer RNA), which is an RNA molecule that carries amino acids to the ribosome during protein synthesis. The large subunit contains three binding sites – the A site, the P site, and the E site.The A site is where the incoming tRNA molecule binds to the mRNA. The P site is where the growing chain of amino acids is held during protein synthesis. The E site is where the spent tRNA molecule exits the ribosome.Function of Ribosomes:Ribosomes are responsible for the synthesis of proteins in the cell. When a ribosome binds to an mRNA molecule, it uses the sequence of codons (three nucleotides) on the mRNA to determine the sequence of amino acids that will be used to build the protein.The ribosome then reads the codons on the mRNA molecule and matches them with specific tRNA molecules that carry the corresponding amino acids. The ribosome then links the amino acids together in the order specified by the mRNA sequence to form a protein.In addition to protein synthesis, ribosomes also play important roles in regulating gene expression and responding to stress conditions in the cell. For example, in response to environmental stress, such as nutrient deprivation or exposure to toxins, cells can alter the composition of their ribosomes, which allows them to produce stress-response proteins that are better suited to the new conditions.Conclusion:In summary, ribosomes are essential components of cells that play a crucial role in protein synthesis. They are made up of ribosomal RNA and proteins and composed of two subunits – a small subunit and a large subunit. Ribosomes read the codons on mRNA and use tRNA molecules to link amino acids together to form proteins. Ribosomes also play important roles in regulating gene expression and responding to stress conditions in the cell.。
稻瘟病菌假定核糖体生成因子MoRei1功能研究

浙江大学学报(农业与生命科学版)48(4):443~452,2022Journal of Zhejiang University (Agric.&Life Sci.)http :///agr E -mail :zdxbnsb @稻瘟病菌假定核糖体生成因子MoRei1功能研究汤帅,徐喆,吕务云,童琪,肖宇,王政逸*(浙江大学生物技术研究所,水稻生物学国家重点实验室,杭州310058)摘要为深入探究稻瘟病菌假定核糖体生成因子MoRei1的生物学功能,通过敲除MoREI1基因、分析ΔMorei1突变体表型、鉴定基因互补及其互作蛋白功能等对其进行了研究。
结果表明:敲除MoREI1基因后,稻瘟病菌ΔMorei1突变体的有性生殖能力丧失,附着胞形成率显著下降,致病力减弱;ΔMorei1突变体对细胞壁和氧化胁迫因子的敏感性增强,附着胞发育过程中糖原的移动和降解速率下降。
稻瘟病菌MoREI1基因可恢复酿酒酵母REI1基因缺失突变体的部分表型,说明MoRei1与酵母核糖体生成因子Rei1在功能上具有同源性,并可能在核糖体生成过程中起类似的作用。
稻瘟病菌MoRei1蛋白与预测的核输出因子MoAlb1间存在物理互作,且MoREI1基因缺失可影响稻瘟病菌MoAlb1蛋白的亚细胞定位。
上述研究结果对阐明MoRei1在稻瘟病菌形态分化和致病性中的作用及其机制有重要意义。
关键词稻瘟病菌;核糖体生成因子MoRei1;功能分析;MoAlb1蛋白中图分类号S 432.1文献标志码A引用格式汤帅,徐喆,吕务云,等.稻瘟病菌假定核糖体生成因子MoRei1功能研究[J].浙江大学学报(农业与生命科学版),2022,48(4):443-452.DOI:10.3785/j.issn.1008-9209.2021.06.153TANG Shuai,XU Zhe,LÜWuyun,et al.Functional analysis of the putative ribosome biogenesis factor MoRei1in Magnaporthe oryzae [J].Journal of Zhejiang University (Agriculture &Life Sciences),2022,48(4):443-452.Functional analysis of the putative ribosome biogenesis factor MoRei1in Magnaporthe oryzaeTANG Shuai,XU Zhe,LÜWuyun,TONG Qi,XIAO Yu,WANG Zhengyi *(State Key Laboratory of Rice Biology,Institute of Biotechnology,Zhejiang University,Hangzhou 310058,China )AbstractTo further explore the biological roles of MoRei1in the rice blast fungus Magnaporthe oryzae ,wecarried out MoREI1gene deletion,phenotypic analysis of ΔMorei1mutants,gene complementation and identification of its interaction proteins.The results showed that the ΔMorei1mutants were defective in sexual reproduction,appressorium formation and pathogenicity.Deletion of MoREI1led to significant increase of sensitivity to cell wall damaging and oxidative stress agents,and delay of glycogen mobilization and degradation during appressorium development.Moreover,the MoREI1gene could partially complement the defects of Saccharomyces cerevisiae Δrei1mutants,indicating that MoRei1is a functional homolog of yeast Rei1,and may share a similar role in ribosome biogenesis.MoRei1physically interacts with MoAlb1,a predicted nuclear export factor.Interestingly,deletion of MoREI1resulted in the impairment of nuclear localization of MoAlb1.These results lay a foundation to clarify the roles of MoRei1in fungal morphogenesis and pathogenicity in M.oryzae.Key wordsMagnaporthe oryzae ;ribosome biogenesis factor MoRei1;functional analysis;MoAlb1proteinDOI :10.3785/j.issn.1008-9209.2021.06.153基金项目:国家自然科学基金项目(31770153,32070141)。
消除评价指标相关性的权值计算方法_李亮

第18卷第2期 2009年4月系统管理学报Journal o f Systems &M anagementVol.18No.2 Apr.2009文章编号:1005-2542(2009)02-0221-05消除评价指标相关性的权值计算方法李 亮, 吴瑞明(上海交通大学安泰经济与管理学院,上海200052)=摘要>根据评价指标的构成要素,提出了一种消除评价指标相关性的代表系权值计算方法。
该方法不改变原有的指标体系,在提出的评价因子概念及其具有的等价性和可加性假设基础上,利用互异代表系理论给出了评价因子贡献值的计算方法,以消除各指标间的相关性。
最后,借助于一个已有案例,说明了代表系权值计算方法的应用步骤,并通过对比分析,表明了该方法的合理性。
关键词:相关性;评价因子;评价指标体系中图分类号:N 94 文献标识码:AA Study on Eliminating Correlation of EvaluationIndex and Obtaining Modified WeightsL I L iang , W U Rui -ming(Antai College o f Economics and M anagement,Shanghai Jiaotong University,Shanghai 200052,China)=Abstract >Based on the constituting elements of evaluatio n index,the paper pr opo ses an alg orithm to e -lim inate cor relation in computing weights of evaluation index.Based on the ex isting ev aluatio n index,w e creativ ely take into consideration an evaluation factor.Then w e propose tw o hy po theses about evaluation facto rs,equivalence and additiv ity.Specifically,w e capture the effects of differing evaluation factors on the evaluatio n index on basis of system of distinct r epresentatives theory.As such,this appro ach generates modified weights by remov ing the correlatio ns betw een assessment attributes.Finally,w ith the help o f an av ailable case,the application procedure of the appro ach is illustrated.By compar ing w ith pr evio us result,the v alidity and efficiency of this alg orithm is justified in the practical applicatio n.Key words:corr elation;evaluation factor;evaluation index sy stem 收稿日期:2008-09-01基金项目:国家自然科学基金资助项目(70671067)作者简介:李 亮(1982-),男,硕士生。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
INTRODUCTION Generating and maintaining a functional proteome is a major challenge for the cell. Defective protein folding often leads to aggregation, which is deleterious for cell viability (Hartl et al., 2011; Tyedmers et al., 2010). Indeed, a growing number of diseases are associated with impaired protein homeostasis (Luheshi et al., 2008; Powers et al., 2009; Voisine et al., 2010). Accordingly, cells contain an array of molecular chaperones, which facilitate protein folding and promote quality control (Hartl et al., 2011; Kramer et al., 2009; Preissler and Deuerling, 2012; Tyedmers et al., 2010). Folding during translation is particularly challenging for the cell. Proteins emerge vectorially from the ribosome exit tunnel
2Department 1Department
SUMMARY
In eukaryotic cells a molecular chaperone network associates with translating ribosomes, assisting the maturation of emerging nascent polypeptides. Hsp70 is perhaps the major eukaryotic ribosomeassociated chaperone and the first reported to bind cotranslationally to nascent chains. However, little is known about the underlying principles and function of this interaction. Here, we use a sensitive and global approach to define the cotranslational substrate specificity of the yeast Hsp70 SSB. We find that SSB binds to a subset of nascent polypeptides whose intrinsic properties and slow translation rates hinder efficient cotranslational folding. The SSBribosome cycle and substrate recognition is modulated by its ribosome-bound cochaperone, RAC. Deletion of SSB leads to widespread aggregation of newly synthesized polypeptides. Thus, cotranslationally acting Hsp70 meets the challenge of folding the eukaryotic proteome by stabilizing its longer, more slowly translated, and aggregation-prone nascent polypeptides.
196 Cell 152, 196–209, January 17, 2013 ª2013 Elsevier Inc.
and cannot fold stably until a domain is fully synthesized (Frydman, 2001). As a result, nascent polypeptide chains are highly susceptible to misfolding and aggregation, particularly given the extreme crowding of the cellular environment (Hartl et al., 2011; Kramer et al., 2009; Preissler and Deuerling, 2012). Eukaryotic and prokaryotic cells have radically different strat` se egies to assist folding of newly translated proteins (Albane et al., 2006; Netzer and Hartl, 1997). In prokaryotic cells, which have a simpler proteome and cell architecture, a single ATPindependent ribosome-bound chaperone, called trigger factor, associates with many nascent chains (Kramer et al., 2009, Oh et al., 2011; Preissler and Deuerling, 2012). Subsequent de novo folding is mostly posttranslational and carried out by the same ATP-dependent chaperones that protect the proteome from stress (Agashe et al., 2004; Hartl and Hayer-Hartl, 2009). In contrast, eukaryotes have developed a complex chaperone network that associates with translating ribosomes and facilitates de novo protein folding. These chaperones linked to protein synthesis comprise several ATP-dependent and ATP-independent factors that are not induced by stress and are coregulated ` se et al., 2006). This is with the translational apparatus (Albane probably due to the fact that eukaryotic proteins are translated with slower kinetics and comprise far more complex architectures than bacterial proteomes (Koonin et al., 2000; Wang et al., 2011). In addition, the compartmentalization of eukaryotic cells necessitates the cotranslational translocation of many proteins into various organelles. Hsp70 is the most prn eukaryotic cells (Frydman, 2001; Kramer et al., 2009). Although it was the first chaperone shown to bind to nascent polypeptides in eukaryotes (Beckmann et al., 1990), its specificity and function in cotranslational protein homeostasis remain undefined. Experiments with model substrates indicate that Hsp70 can bind to nascent chains early in translation and facilitate their folding, translocation, or association with downstream chaperones (Frydman and Hartl, 1996; Frydman et al., 1994; Hartl and Hayer-Hartl, 2009). Hsp70s are highly conserved and ubiquitous ATP-dependent chaperones implicated in various key aspects of protein homeostasis (Kampinga and Craig, 2010). In yeast, the cytosolic
