编译原理第4章作业答案
编译原理-第4章 语法分析--习题答案

第4章语法分析习题答案1.判断(1)由于递归下降分析法比较简单,因此它要求文法不必是LL(1)文法。
(× )LL(1)文法。
(× )(3)任何LL(1)文法都是无二义性的。
(√)(4)存在一种算法,能判定任何上下文无关文法是否是LL(1) 文法。
(√)(× )(6)每一个SLR(1)文法都是LR(1)文法。
(√)(7)任何一个LR(1)文法,反之亦然。
(× )(8)由于LALR是在LR(1)基础上的改进方法,所以LALR(× )(9)所有LR分析器的总控程序都是一样的,只是分析表各有不同。
(√)(10)算符优先分析法很难完全避免将错误的句子得到正确的归约。
(√)2.文法G[E]:E→E+T|TT→T*F|FF→(E)|i试给出句型(E+F)*i的短语、简单短语、句柄和最左素短语。
答案:画出语法树,得到:短语: (E+F)*i ,(E+F) ,E+F ,F ,i简单短语: F ,i句柄: F最左素短语: E+F3.文法G[S]:S→SdT | TT→T<G | GG→(S) | a试给出句型(SdG)<a的短语、简单短语、句柄和最左素短语。
答案:画出语法树,得到:短语:(SdG)<a 、(SdG) 、SdG 、G 、a简单(直接)短语:G 、a句柄:G最左素短语:SdG4.对文法G[S]提取公共左因子进行改写,判断改写后的文法是否为LL(1)文法。
S→if E then S else SS→if E then SS→otherE→b答案:提取公共左因子;文法改写为:S→if E then S S'|otherS'→else S|E→bLL(1)文法判定:① 文法无左递归② First(S)={if,other}, First(S')={else, }First(E)={b}Follow(S)= Follow(S')={else,#}Follow(E)={then}First(if E then S S')∩First(other)=First(else S)∩First( )=③First(S')∩Follow(S')={else}不为空集故此文法不是LL(1)文法。
编译基础学习知识原理第4章规范标准答案
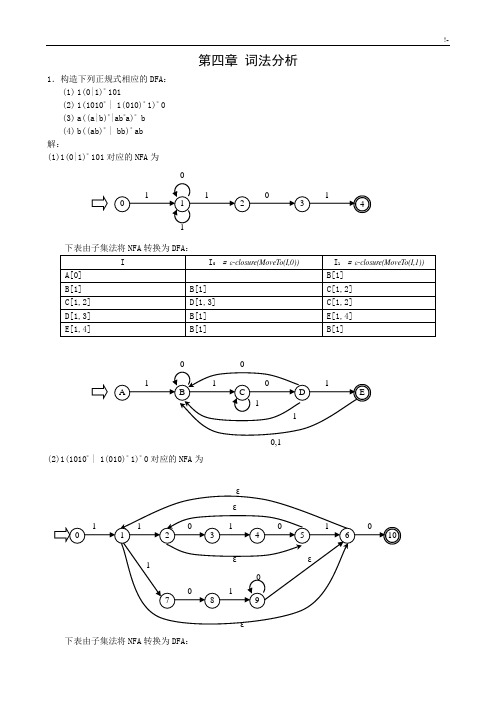
第四章 词法分析1.构造下列正规式相应的DFA :(1) 1(0|1)*101(2) 1(1010* | 1(010)* 1)*(3) a((a|b)*|ab *a)*b(4) b((ab)* | bb)*ab 解:(1)1(0|1)*101对应的NFA 为下表由子集法将NFA 转换为DFA :(2)1(1010* | 1(010)* 1)*0对应的NFA 为下表由子集法将NFA 转换为DFA :(3)a((a|b)*|ab*a)* b (略)(4)b((ab)* | bb)* ab (略)2.已知NFA=({x,y,z},{0,1},M,{x},{z})其中:M(x,0)={z},M(y,0)={x,y},M(z,0)={x,z},M(x,1)={x}, M(y,1)=φ,M(z,1)={y},构造相应的DFA。
解:根据题意有NFA图如下0,1 下表由子集法将NFA转换为DFA:下面将该DFA最小化:(1)首先将它的状态集分成两个子集:P1={A,D,E},P2={B,C,F}(2)区分P2:由于F(F,1)=F(C,1)=E,F(F,0)=F并且F(C,0)=C,所以F,C等价。
由于F(B,0)=F(C,0)=C,F(B,1)=D,F(C,1)=E,而D,E不等价(见下步),从而B与C,F可以区分。
有P21={C,F},P22={B}。
(3)区分P1:由于A,E输入0到终态,而D输入0不到终态,所以D与A,E可以区分,有P11={A,E},P12={D}。
(4)由于F(A,0)=B,F(E,0)=F,而B,F不等价,所以A,E可以区分。
(5)综上所述,DFA可以区分为P={{A},{B},{D},{E},{C,F}}。
所以最小化的DFA如下:3.将图4.16确定化:图4.16解:下表由子集法将NFA转换为DFA:14.把图4.17的(a)和(b)分别确定化和最小化:(a) (b)解: (a):可得图(a1),由于F(A,b)=F(B,b)=C,并且F(A,a)=F(B,a)=B,所以A,B 等价,可将DFA 最小化,即:删除B ,将原来引向B 的引线引向与其等价的状态A ,有图(a2)。
编译原理第4章习题答案
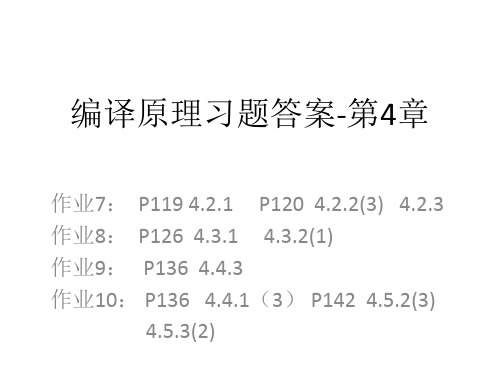
S-> S’ S’->(S)SS’| First( (S)SS’) = { ( } Follow(S)=Follow(S’)= { (, ),$ }
预测分析表
非终结符 S ( S->S’ ) S->S’ $ S->S’
S’
S’->(S)SS’
S’->
S’->
S’->
冲突
仔细分析后,发现该文法 S->S(S)S| 是二义性文法。 二义性文法不可能是LL(1)文法。 例如:( ) ( )
S->aS’ S’->aS’AS’|Ɛ A->+|*
First(S) = First(aS’)={a} First(S’)= First(aS’AS’) ∪ First(Ɛ)= {a} ∪{Ɛ}= {a, Ɛ} First(A) = { +,*}
Follow(S) ={$} 因为 S->aS’,所以把Follow(S)加入到Follow(S’)中。 因为 S’->aS’AS’的第一个S’ ,所以把First(AS’)={+,*}加入到Follow(S’)中。 因为 S’->aS’AS’的第二个S’ ,所以Follow(S)加入到Follow(S’)中。 所以,Follow(S’)= {$, +,*} 对 S’->aS’AS’的A ,当A后面的S’推导出非空时,把First(S’)-{Ɛ}={a}加入到Follow(A)中。 对 S’->aS’AS’的A ,当A后面的S’推导出空时,把Follow(S’)={$,+,*}加入到Follow(A)中。 所以,Follow(A)= {a, +,*,$} 对于S’->aS’AS’|Ɛ,因为First(aS’AS’) ∩Follow(S’)={a} ∩{$,+,*}=空集,所以没有冲突。 对于A->+|*,因为First(+) ∩First(*)={+} ∩{*}=空集,所以没有冲突。 所以该文法是LL(1)文法。
蒋立源编译原理第三版第四章习题与答案.docx

第五章习题5-1设有文法G[S] :S→A/A→aA∣ AS∣/(1)找出部分符号序偶间的简单优先关系。
(2)验证 G[S] 不是简单优先文法。
5-2对于算符文法G[S] :S→E E→ E-T∣ T T→T*F∣F F→ -P∣P P→ (E)∣i(1)找出部分终结符号序偶间的算符优先关系。
(2)验证 G[S] 不是算符优先文法。
5-3 设有文法G′[E] :E→E E→E+T |T1T →T T→T*F|F F→(E)|i11111其相应的简单优先矩阵如题图5-3所示,试给出对符号串(i+i )进行简单优先分析的过程。
题图 5-3 文法 G′ [E] 的简单优先矩阵5-4设有文法G[E]:E→E+T|TT→T*F|FF→(E)|i其相应的算符优先矩阵如题图5-4 所示。
试给出对符号串(i+i )进行算符优先分析的过程。
(i*+)#(○○○○○<<<<=i○○○○>>>>○○○○○○*<<>>>>○○○○○○+<<<>>>○○○○)>>>>○○○○#<<<<题图 5-4文法 G[E] 的算符优先矩阵5-5对于下列的文法,试分别构造识别其全部可归前缀的DFA和 LR(0) 分析表,并判断哪些是LR(0) 文法。
(1)S →aSb∣ aSc∣ ab(2)S →aSSb∣ aSSS∣c(3)S →AA→Ab∣ a5-6下列文法是否是SLR(1)文法?若是,构造相应的SLR(1) 分析表,若不是,则阐明其理由。
(1) S →Sab∣ bR R→S∣a(2) S →aSAB∣ BA A→aA∣ B B→ b(3) S →aA∣bB A→cAd∣εB→cBdd∣ε5-7对如下的文法分别构造LR(0) 及 SLR(1) 分析表,并比较两者的异同。
S→cAd∣ b A→ASc∣a5-8对于文法G[S]:S→A A→BA∣εB→ aB∣ b(1)构造 LR(1) 分析表;(2)给出用LR(1)分析表对输入符号串abab 的分析过程。
编译原理课后习题答案ch4

注意:本题应该理解为对图(a)进行确定化和对图(b)进行最小化。提供的答案没有对图(a)确 定化。
盛威网()专业的计算机学习网站 14
《编译原理》课后习题答案第四章
第5题 构造一个 DFA,它接收 Σ={0,1}上所有满足如下条件的字符串:每个 1 都有 0 直接跟在 右边。并给出该语言的正规式。 答案: 按题意相应的正规表达式是(0*10)*0*, 或 0*(0 | 10)*0* 构造相应的 DFA, 首先构造 NFA 为
7
《编译原理》课后习题答案第四章
注意:这个题,也可以这样构造 NFA(用最少的ε,但注意不能出错) :
a,b 0 a a 2 a,b 1 a b 3
盛威网()专业的计算机学习网站
8
《编译原理》课后习题答 ε X b A ε F B a C b ε b G b H ε ε D ε E a I b Y
除 X,A 外,重新命名其他状态,令 AB 为 B、AC 为 C、ABY 为 D,因为 D 含有 Y(NFA 的终态),所以 D 为终态。 . X A B C D DFA 的状态图:: 0 . A C A C 1 A B B D B
(2)先构造 NFA: 0 ε X 1 A ε ε F B 1 C 1 0 ε 0 D 1 E 1 0 ε 用子集法将 NFA 确定化 ε X T0=X A T1= ABFL Y CG T2= Y T3= CGJ DH K T4= DH EI T5= ABFKL T6= ABEFIL EJY T7= ABEFGJLY EHY CGK T8= ABEFHLY EY CGI T9= ABCFGJKL DHY T10= ABEFLY T11= CGJI DHJ T12= DHY T13= DHJ EIK T14= ABEFIKL ABEFIKL EJY CG DHJ ?正确 DHJG EI EIK DHY EY DHJ CG K ABEFLY CGJI DHY CGK ABEFHLY ABCFGJKL EY CGI ABEFGJLY EHY CGK ABEFIL Y EJY CG CG DH ABFKL EI DH K Y CGJ ABFL Y CG X A 0 1 1 ε L ε K ε J 0 Y
编译原理第四章参考答案

编译原理第四章参考答案1.1考虑下⾯⽂法G1S->a|^|(T)T->T,S|S消去G1的左递归。
然后对每个⾮终结符,写出不带回溯的递归⼦程序。
答::(1)消除左递归:S->a|^|(T)T-> ST’T’->,S T’|ε(2)first(S)={ a , ^ , ( } first(T)= { a , ^ , ( } first(T’)={ , ε}First(a)={a},First(^)={^},First( (T) )={ ( }S的所有候选的⾸符集不相交First(,ST’)={,} ,First(ε)={ε},T’的所有候选的⾸符集不相交Follow(T’)=Follow(T)={ )}first(T’)∩Follow(T’)={}所以改造后的⽂法为LL(1)⽂法。
不带回溯的递归⼦程序如下:S( ){if (lookahead=’a’) advance;Else if(lookahead=’^’) advance;Else if(lookahead=’(’){advance;T();if(lookahead=’)’) advance;else error();}Else error();}T( ){S( );T’( ):}T’->,S T’|εT’( ){if (lookahead=’,’){advance;T’();}Else if(lookahead=Follow(T’)) advance;Else error;}有⽂法G(S):S→S+aF|aF|+aFF→*aF|*a(1)改写⽂法为等价⽂法G[S’],消除⽂法的左递归和回溯(2)构造G[S’]相应的FIRST和FOLLOW集合;(3)构造G[S’]的预测分析表,以此说明它是否为LL(1)⽂法。
(4)如果是LL(1)⽂法,请给出句⼦a*a+a*a*a的预测分析过程该⽂法为LL(1)⽂法,因为它的预测分析表中⽆冲突项。
编译原理第4章答案

第四章 词法分析1.构造下列正规式相应的DFA :(1) 1(0|1)*101(2) 1(1010*| 1(010)*1)*0 (3) a((a|b)*|ab *a)*b (4) b((ab)*| bb)*ab 解:(1)1(0|1)*101对应的NFA 为下表由子集法将NFA 转换为DFA :(2)1(1010*| 1(010)*1)*0对应的NFA 为 10,1下表由子集法将NFA转换为DFA:(3)a((a|b)*|ab *a)*b (略) (4)b((ab)*| bb)*ab (略)2.已知NFA=({x,y,z},{0,1},M,{x},{z})其中:M(x,0)={z},M(y,0)={x,y},M(z,0)={x,z},M(x,1)={x}, M(y,1)=φ,M(z,1)={y},构造相应的DFA 。
解:根据题意有NFA 图如下下表由子集法将NFA 转换为DFA :0,1下面将该DFA最小化:(1)首先将它的状态集分成两个子集:P1={A,D,E},P2={B,C,F}(2)区分P2:由于F(F,1)=F(C,1)=E,F(F,0)=F并且F(C,0)=C,所以F,C等价。
由于F(B,0)=F(C,0)=C,F(B,1)=D,F(C,1)=E,而D,E不等价(见下步),从而B与C,F可以区分。
有P21={C,F},P22={B}。
(3)区分P1:由于A,E输入0到终态,而D输入0不到终态,所以D与A,E可以区分,有P11={A,E},P12={D}。
(4)由于F(A,0)=B,F(E,0)=F,而B,F不等价,所以A,E可以区分。
(5)综上所述,DFA可以区分为P={{A},{B},{D},{E},{C,F}}。
所以最小化的DFA如下:3.将图确定化:1101111解:下表由子集法将NFA 转换为DFA :4.把图的(a)和(b)分别确定化和最小化:(a) (b)解: (a):下表由子集法将NFA 转换为DFA :0,1a可得图(a1),由于F(A,b)=F(B,b)=C,并且F(A,a)=F(B,a)=B,所以A,B 等价,可将DFA 最小化,即:删除B ,将原来引向B 的引线引向与其等价的状态A ,有图(a2)。
编译原理与实践第四章答案

The exercises of Chapter Four4.2Grammar: A → ( A ) A | εAssume we have lookahead of one token as in the example on p. 144 in the text book. Procedure A()if (LookAhead() ∈{‘(‘}) thenCall Expect(‘(‘)Call A()Call Expect (‘)’)Call A()elseif (LookAhead()∈{‘)‘, $}) thenreturn()else/* error */fifiend4.3 Given the grammarstatement→assign-stmt|call-stmt|otherassign-stmt→identifier:=expcall-stmt→identifier(exp-list)[Solution]First, convert the grammar into following forms:statement→identifier:=exp | identifier(exp-list)|otherThen, the pseudocode to parse this grammar:Procedure statementBeginCase token of( identifer : match(identifer);case token of( := : match(:=);exp;( (: match(();exp-list;match());else error;endcase(other: match(other);else error;endcase;end statement4.7 aGrammar: A → ( A ) A | εFirst(A)={(,ε } Follow(A)={$,)}4.7 bSee theorem on P.178 in the text book1.First{(}∩First{ε}=Φ2.ε∈Fist(A), First(A) ∩Follow(A)= Φboth conditions of the theorem are satisfied, hence grammar is LL(1)4.9 Consider the following grammar:lexp→atom|listatom→number|identifierlist→(lexp-seq)lexp-seq→lexp, lexp-seq|lexpa. Left factor this grammar.b. Construct First and Follow sets for the nonterminals of the resulting grammar.c. Show that the resulting grammar is LL(1).d. Construct the LL(1) parsing table for the resulting grammar.e. Show the actions of the corresponding LL(1) parser, given the input string (a,(b,(2)),(c)). [Solution]a.lexp→atom|listatom→number|identifierlist→(lexp-seq)lexp-seq→lexp lexp-seq’lexp-seq’→, lexp-seq|εb.First(lexp)={number, identifier, ( }First(atom)={number, identifier}First(list)={( }First(lexp-seq)={ number, identifier, ( }First(lexp-seq’)={, , ε}Follow(lexp)={, $, } }Follow(atom)= {, $, } }Follow(list)= {, $, } }Follow(lexp-seq)={$, } }Follow(lexp-seq’)={$,} }c. According to the defination of LL(1) grammar (Page 155), the resulting grammar is LL(1) as each table entry has at most one production as shown in (d).4.10 aLeft factored grammar:1. decl → type var-list2. type → int3. type → float4. var-list → identifier B5. B → , var-list6. B→ ε4.10 b4.10 c4.10 e4.12 a. Can an LL(1) grammar be ambigous? Why or Why not?b. Can an ambigous grammar be LL(1)? Why or Why not?c. Must an unambigous grammar be LL(1)? Why or Why not?[Solution]Defination of an ambiguous grammar: A grammar that generates a string with two distinct parse trees. (Page 116)Defination of an LL(1) grammar: A grammar is an LL(1) grammar if the associatied LL(1) parsing table has at most one priduction in each table entry.a.An LL(1) grammar can not be ambiguous, since the defination implies that an unambiguous parse canbe constructed using the LL(1) parsing tableb.An ambiguous grammar can not be LL(1) grammar, but can be convert to be ambiguous by usingdisambiguating rule.c.An unambiguous grammar may be not an LL(1) grammar, since some ambiuous grammar can beparsed using LL(K) table, where K>1.4.13Left recursive grammars can not be LL(1):Consider a grammar with a production A →A βwith symbol t in its first set. IF A is on the top of the stack and the current look-ahead token is t, A will be popped off the stack and then βwill be pushed onto the stack followed by A. A will again be the top of the stack and the look-ahead token will still be t, so it will repeat again, and again, infinitely, or until you get a stack overflow.Note: The first set for the recursive production will have a non-empty intersection with the first set for the terminating production, which will lead to a conflict when creating the LL(1) table.。
编译原理第四章作业答案

非终结符
FIRSTVT
LASTVT
E
+ * ↑( i
+ * ↑) i
T
* ↑( i
* ↑) i
F
↑( i
↑)i
P
(i
)i
2).关系 1.由#E#,知 # = # ;由(E)知 ( = ) 2.求 < 关系 考察对象:文法中终结符号在前,非终结符号在后的相邻符号对 由#E # < FIRSTVT(E) 由+T + < FIRSTVT(T) 由*F * < FIRSTVT(F) 由↑F ↑< FIRSTVT(F) 由(E ( < FIRSTVT(E)
T→F
F→-P
F→P
P→(E)
P→i
(1) 构造 G 的算符优先矩阵;
(2) 指出 G 不是算符优先文法,即指出具有多重定义的优先矩阵元素;
(3)将 G 改写为算符优先文法。
解:
(1)求每个非终结符号的 FIRSTVT 集和 LASTVT 集
S→E
E→E-T|T
T→T*F|F
F→-P|P
P→(E)|i
Z 11 Z 12 Z 13
(S
A
B ) = (φ (S ) + () [S ] + [])Z 21
Z 22
Z
23
Z 31 Z 32 Z 33
Z 11 Z 12 Z 13 ε φ φ A B φ Z 11 Z 12 Z 13
Z 21
Z 22
Z
23
=
φ
ε
φ
+
ε
φ
φ
Z
21
Z 22
编译原理第4章习题解答

第4章习题解答:1,2,3,4 解答略!5. 解答:(1)× (2)√ (3)× (4)√ (5)√ (6)√(7)×(8)×6. 解答:(1)A:④ B:③ C:③ D:④ E:②(2)A:④ B:④ C:③ D:③ E:②7.解答:(1) 消除给定文法中的左递归,并提取公因子:bexpr→bterm {or bterm }bterm→bfactor {and bfactor}bfactor→not bfactor | (bexpr) | true |false(2) 用类C语言写出其递归分析程序:void bexpr();{bterm();WHILE(lookahead =='or') { match ('or');bterm();}}void bterm();{bfactor();WHILE(lookahead =='and'){ match ('and');bfactor();}} void bfactor();{if (llokahead=='not') then {match ('not');bfactor();}else if(lookahead=='(') then {match (‘(');bexpr();match(')');}else if(lookahead =='true')then match ('true) else if (lookahead=='false')then match ('false');else error;}8. 解答:消除所给文法的左递归,得G':S →(L)|aL → SL'L'→ ,SL' |实现预测分析器的不含递归调用的一种有效方法是使用一张分析表和一个栈进行联合控制,下面构造预测分析表:根据文法G'有:First(S) = { ( , a ) Follow(S) = { ) , , , #}First(L) = { ( , a ) Follow(L) = { ) }First(L') = { ,} Follow(L') = { ) }按以上结果,构造预测分析表M如下:文法G'是LL(1)的,因为它的LL(1)分析表不含多重定义入口。
编译原理教程课后习题答案——第四章

第四章语义分析和中间代码生成4.1 完成下列选择题:(1) 四元式之间的联系是通过实现的。
a. 指示器b. 临时变量c. 符号表d. 程序变量(2) 间接三元式表示法的优点为。
a. 采用间接码表,便于优化处理b. 节省存储空间,不便于表的修改c. 便于优化处理,节省存储空间d. 节省存储空间,不便于优化处理(3) 表达式(┐A∨B)∧(C∨D)的逆波兰表示为。
a. ┐AB∨∧CD∨b. A┐B∨CD∨∧c. AB∨┐CD∨∧d. A┐B∨∧CD∨(4) 有一语法制导翻译如下所示:S→bAb {print″1″}A→(B {print″2″}A→a {print″3″}B→Aa) {print″4″}若输入序列为b(((aa)a)a)b,且采用自下而上的分析方法,则输出序列为。
a. 32224441 b. 34242421c. 12424243d. 34442212【解答】(1) b (2) a (3) b (4) b4.2 何谓“语法制导翻译”?试给出用语法制导翻译生成中间代码的要点,并用一简例予以说明。
【解答】语法制导翻译(SDTS)直观上说就是为每个产生式配上一个翻译子程序(称语义动作或语义子程序),并且在语法分析的同时执行这些子程序。
也即在语法分析过程中,当一个产生式获得匹配(对于自上而下分析)或用于归约(对于自下而上分析)时,此产生式相应的语义子程序进入工作,完成既定的翻译任务。
用语法制导翻译(SDTS)生成中间代码的要点如下:(1) 按语法成分的实际处理顺序生成,即按语义要求生成中间代码。
(2) 注意地址返填问题。
(3) 不要遗漏必要的处理,如无条件跳转等。
例如下面的程序段:if (i>0) a=i+e-b*d; else a=0;在生成中间代码时,条件“i>0”为假的转移地址无法确定,而要等到处理“else”时方可确定,这时就存在一个地址返填问题。
此外,按语义要求,当处理完(i>0)后的语句(即“i>0”为真时执行的语句)时,则应转出当前的if语句,也即此时应加入一条无条件跳转指令,并且这个转移地址也需要待处理完else之后的语句后方可获得,就是说同样存在着地址返填问题。
编译原理与实践 第四章 答案

The exercises of Chapter Four4、2Grammar: A → ( A ) A | εAssume we have lookahead of one token as in the example on p、144 in the text book、Procedure A()if (LookAhead() ∈{‘(‘}) thenCall Expect(‘(‘)Call A()Call Expect (‘)’)Call A()elseif (LookAhead()∈{‘)‘, $}) thenreturn()else/* error */fifiend4、3 Given the grammarstatement→assign-stmt|call-stmt|otherassign-stmt→identifier:=expcall-stmt→identifier(exp-list)[Solution]First, convert the grammar into following forms:statement→identifier:=exp | identifier(exp-list)|otherThen, the pseudocode to parse this grammar:Procedure statementBeginCase token of( identifer : match(identifer);case token of( := : match(:=);exp;( (: match(();exp-list;match());else error;endcase(other: match(other);else error;endcase;end statement4、7 aGrammar: A → ( A ) A | εFirst(A)={(,ε } Follow(A)={$,)}4、7 bSee theorem on P、178 in the text book1.First{(}∩First{ε}=Φ2.ε∈Fist(A), First(A) ∩Follow(A)= Φboth conditions of the theorem are satisfied, hence grammar is LL(1)4、9 Consider the following grammar:lexp→atom|listatom→number|identifierlist→(lexp-seq)lexp-seq→lexp, lexp-seq|lexpa、Left factor this grammar、b、Construct First and Follow sets for the nonterminals of the resulting grammar、c、Show that the resulting grammar is LL(1)、d、Construct the LL(1) parsing table for the resulting grammar、e、Show the actions of the corresponding LL(1) parser, given the input string (a,(b,(2)),(c))、[Solution]a、lexp→atom|listatom→number|identifierlist→(lexp-seq)lexp-seq→lexp lexp-seq’lexp-seq’→, lexp-seq|εb、First(lexp)={number, identifier, ( }First(atom)={number, identifier}First(list)={( }First(lexp-seq)={ number, identifier, ( }First(lexp-seq’)={, , ε}Follow(lexp)={, $, } }Follow(atom)= {, $, } }Follow(list)= {, $, } }Follow(lexp-seq)={$, } }Follow(lexp-seq’)={$,} }c、According to the defination of LL(1) grammar (Page 155), the resulting grammar is LL(1) as each table entry has at most one production as shown in (d)、de4、10 aLeft factored grammar:1、decl → type var-list2、type → int3、type → float4、var-list → identifier B5、B → , var-list6、B→ ε4、10 b4、10 cM[N, T] int float identifier , $decl 1 1type 2 3var-list 4B 5 6Parsing stack Input Action$ decl int x, y, z $ decl →type var-list $ var-list type int x, y, z $ type →int$ var-list int int x, y, z $ match int$ var-list x, y, z $ var-list →identifer B $ B identifier x, y, z $ match identifier w/ x $ B , y, z $ B →, var-list$ var-list , , y, z $ match ,$ var-list y, z $ var-list →identifer B $ B identifier y, z $ match identifier w/ y $ B , z $ B →, var-list$ var-list , , z $ match ,$ var-list z $ var-list →identifer B $ B identifier z $ match identifier w/ z4、12 a、Can an LL(1) grammar be ambigous? Why or Why not?b、Can an ambigous grammar be LL(1)? Why or Why not?c、Must an unambigous grammar be LL(1)? Why or Why not?[Solution]Defination of an ambiguous grammar: A grammar that generates a string with two distinct parse trees、(Page 116)Defination of an LL(1) grammar: A grammar is an LL(1) grammar if the associatied LL(1) parsing table has at most one priduction in each table entry、a.An LL(1) grammar can not be ambiguous, since the defination implies that an unambiguous parse canbe constructed using the LL(1) parsing tableb.An ambiguous grammar can not be LL(1) grammar, but can be convert to be ambiguous by usingdisambiguating rule、c.An unambiguous grammar may be not an LL(1) grammar, since some ambiuous grammar can beparsed using LL(K) table, where K>1、4、13Left recursive grammars can not be LL(1):Consider a grammar with a production A →A βwith symbol t in its first set、IF A is on the top of the stack and the current look-ahead token is t, A will be popped off the stack and then βwill be pushed onto the stack followed by A、A will again be the top of the stack and the look-ahead token will still be t, so it will repeat again, and again, infinitely, or until you get a stack overflow、Note: The first set for the recursive production will have a non-empty intersection with the first set for the terminating production, which will lead to a conflict when creating the LL(1) table、。
编译原理 第4章习题解答

第四章习题解答4.1词法分析的主要任务是对源程序进行扫描,从中识别出单词,它是编译过程的第一步,也是编译过程中不可缺少的部分。
4.2单词符号一般分为五类:关键字、标识符、常数、运算符和界限符。
分别用整数1、2、3、4、5表示。
对于这种非一符一个类别码的编码形式,一个单词符号除了给出它的单词类别码之外,还要给出它的自身值。
标识符的自身值被表示成按字节划分的内部码。
常数的自身值是其二进制值。
由于语言中的关键字、运算符和界限符的数量都是确定的,因此,对这些单词符号可采用一符一个单词类别码。
如果采取一符一个单词类别码,那么这些单词符号的自身值就不必给出了。
4.3设计词法分析程序有如下几种方法:①由正规文法设计词法分析程序——程序设计语言的单词一般都可以用正规文法描述,从正规文法可构造一个FA。
再对FA确定化和状态个数最少化,最后得到一个化简了的DFA。
这个DFA正是词法分析程序的设计框图,这样,由DFA编制词法分析程序就容易了。
②由正规表达式设计词法分析程序——正规表达式也是描述单词的一种方便工具。
由正规表达式转换成NDFA,然后再对它确定化和状态个数最少化,可得一个DFA。
再由DFA编制词法分析程序。
4.4符号表在编译程序中具有十分重要的意义,它是编译程序中不可缺少的部分。
在编译程序中,符号表用来存放在程序中出现的各种标识符及其语义属性。
一个标识符包含了它全部的语义属性和特征。
标识符的全部属性不可能在编译程序的某一个阶段获得,而需要在它的各个阶段中去获得。
在编译程序的各个阶段,不仅要用获取的标识符信息去更新符号表中的内容,添加新的标识符及其属性,而且需要去查找符号表,引用符号表中的信息。
因为,符号表是编译程序进行各种语义检查(即语义分析)的依据,是进行地址分配的依据。
标识符处理的基本思想是,当遇到定义性标识符时,先去查符号表(标识符表)。
如果此标识符已在符号表中登记过,那么表明该标识符被多次声明,将作为一个错误,因为一个标识符只能被声明一次;如果标识符在符号表中未登记过,那么将构造此标识符的机内符,并在符号表中进行登记。
编译原理第4章答案

编译原理第4章答案第四章词法分析1.构造下列正规式相应的DFA :(1) 1(0|1)* 101 (2) 1(1010* | 1(010)* 1)* 0 (3) a((a|b)*|ab *a)* b (4) b((ab)* | bb)*ab 解:(1)1(0|1)*101对应的NFA 为(2)1(1010* | 1(010)* 1)* 0对应的NFA 为下表由⼦集法将NFA 转换为DFA :0 0,1(3)a((a|b)*|ab*a)* b (略)(4)b((ab)* | bb)* ab (略)2.已知NFA=({x,y,z},{0,1},M,{x},{z})其中:M(x,0)={z},M(y,0)={x,y},M(z,0)={x,z},M(x,1)={x}, M(y,1)=φ,M(z,1)={y},构造相应的DFA。
解:根据题意有NFA图如下0,1 下表由⼦集法将NFA转换为DFA:下⾯将该DFA最⼩化:(1)⾸先将它的状态集分成两个⼦集:P1={A,D,E},P2={B,C,F}(2)区分P2:由于F(F,1)=F(C,1)=E,F(F,0)=F并且F(C,0)=C,所以F,C等价。
由于F(B,0)=F(C,0)=C, F(B,1)=D,F(C,1)=E,⽽D,E不等价(见下步),从⽽B与C,F可以区分。
有P21={C,F},P22={B}。
(3)区分P1:由于A,E输⼊0到终态,⽽D输⼊0不到终态,所以D与A,E可以区分,有P11={A,E},P12={D}。
(4)由于F(A,0)=B,F(E,0)=F,⽽B,F不等价,所以A,E可以区分。
(5)综上所述,DFA可以区分为P={{A},{B},{D},{E},{C,F}}。
所以最⼩化的DFA如下:3.将图4.16确定化:图4.16解:下表由⼦集法将NFA转换为DFA:14.把图4.17的(a)和(b)分别确定化和最⼩化:(a) (b)解: (a):下表由⼦集法将NFA 转换为DFA :可得图(a1),由于F(A,b)=F(B,b)=C,并且F(A,a)=F(B,a)=B,所以A,B 等价,可将DFA 最⼩化,即:删除B ,将原来引向B 的引线引向与其等价的状态A ,有图(a2)。
蒋立源编译原理第三版第四章-习题与答案1

第4章习题14-1 消除以下文法的左递归性。
(1) S→SA|AA→SB|B|(S)|( ) B→[S]|[ ](2) S→AS|b A→SA|a(3) S→(T)|a|ε T→S|T,S4-2 对于如下文法,求各候选式的FIRST集和各非终结符号的FOLLOW集。
S→aAB|bA|ε A→aAb|ε B→bB|ε4-3 验证以下文法是否为LL(1)文法。
(1) S→AB|CDa A→ab|c B→dE|εC→eC|εD→fD|fE→dE|ε(2) S→aABbCD|ε A→ASd|ε B→SAc|eC|εC→Sf|Cg|εD→aBD|ε4-4 对于如下的文法G[S]:S→Sb|Ab|bA→Aa|a(1)构造一个与G等价的LL(1)文法G′[S];(2)对于G′[S],构造相应的LL(1)分析表;(3) 利用LL(1)分析法判断符号串aabb是否是文法G[S]的合法句子。
4-5 设已给文法S→SaB|bB A→S|a B→Ac(1) 构造一个与G等价的LL(1)文法G′[S];(2) 对于G′[S],构造相应的LL(1)分析表;(3) 利用LL(1)分析法判断符号串bacabc是否是文法G[S]的合法句子。
第4章习题答案4-1解:(1)文法G[S]中的S,A都是间接左递归的非终结符号。
将A产生式的右部代入产生式S→A中,得到与原文法等价的文法G′[S]:S→SA|SB|B|(S)|( )A→SB|B|(S)|( )B→[S]|[ ]文法G′[S]中的S是直接左递归的非终结符号,则消除S产生式的直接递归性后,我们便得到了与原文法等价且无任何左递归性的文法G"[S]:S→BS′|(S)S′|( )S′S′→AS′|BS′|εA→SB|B|(S)|( )B→[S]|[ ](2)文法G[S]中的S,A都是间接左递归的非终结符号。
将A产生式代入产生式S→AS中,得到与原文法等价的文法G′[S]:S→SAS|aS|bA→SA|a文法G′[S]中的S是直接左递归的非终结符号,则消除S产生式的直接递归性后,我们便得到了与原文法等价且无任何左递归性的文法G"[S]:S→aSS′|bS′S′→ASS′|εA→SA|a(3)文法G[S]中的T是直接左递归的非终结符号。
编译原理英文版第四章答案

Chapter 4 Answers - Compiler Principles Exercise 1Question:Consider the following context-free grammar (CFG):S → a | BB → a1.Is the language generated by this CFG ambiguous? Justify your answer.2.If the CFG is ambiguous, provide a string that can be generated in twodifferent ways.Answer:1.The language generated by this CFG is not ambiguous. In order toprove this, we need to show that for every valid string in the language, there isa unique deriv ation tree. Let’s analyze the grammar to determine thisuniqueness:•The production rule S → a is a direct derivation from the start symbol S, resulting in the terminal symbol a. Since this is the only possible derivation from S, it is unambiguous.•The production rule S → B has B as its right-hand side. The production rule B → a is the only production rule for B, so B will eventually derive theterminal a. Therefore, the derivation from S to the single terminal a is unique.Hence, we can conclude that the language generated by this CFG is not ambiguous because there is a unique derivation for every valid string.2.Since the language is not ambiguous, we cannot provide a string thatcan be generated in two different ways, as every valid string has only onederivation.Exercise 2Question:Consider the following context-free grammar (CFG):E → E + E | E * E | id1.Starting with the non-terminal symbol E, apply the productions of thegrammar to generate the string id + id * id.2.Draw a parse tree for the string id + id * id using the abovegrammar.Answer:1.To generate the string id + id * id, we can apply the productions ofthe grammar as follows:E (Starting with E)E + E (Using E → E + E)E + E * E (Using E → E * E)id + id * id (Using E → id)2.The parse tree for the string id + id * id is as follows:E_____|_____| | |E + E| |E E| |id __|__| |E *| |E id|idExercise 3Question:Provide a context-free grammar (CFG) for the language of palindromes over the alphabet {a, b}.Answer:We can define a context-free grammar (CFG) to generate palindromes over the alphabet {a, b} as follows:S → ε | a | b | aSa | bSbExplanation: - The production rule S → ε generates an empty string (epsilon). - The production rules S → a and S → b generate single characters a and b respectively. - The production rules S → aSa and S → bSb generate palindromes. Here, the recursive non-terminal S is enclosed between the same terminal symbols a or b.This CFG covers all possible palindromes over the alphabet {a, b}, including empty strings, single characters, and palindromic strings of various lengths.Exercise 4Question:Consider the following context-free grammar (CFG):S → AaBA → ε | aAB → ε | bB1.What is the language generated by this CFG?2.Determine if the CFG is ambiguous. If so, provide a string that can begenerated in two different ways.Answer:1.The language generated by this CFG consists of strings with a prefix ofa and a suffix of b. The generated language can be represented as:L = {anbm | n ≥ 0, m ≥ 0}Where an represents zero or more a and bn represents zero or more b.2.The CFG is ambiguous. Here is an example of a string that can begenerated in two different ways: aab.Possible derivations:•Derivation 1:S (Start with S)AaB (Using S → AaB)aaABb (Using A → aA)aaBb (Using A → ε)aab (Using B → bB)•Derivation 2:S (Start with S)AaB (Using S → AaB)AaaBBb (Using A → aA)aaBBb (Using A → ε)aaBb (Using B → ε)aab (Using B → bB)Thus, the string aab can be derived in two different ways, making the grammar ambiguous.Exercise 5Question:Consider the following context-free grammar (CFG):E → E + D | DD → D * F | FF → idStarting with the non-terminal symbol E, apply the productions of the grammar to generate the string id + id * id.Answer:To generate the string id + id * id, we can apply the productions of the grammar as follows:E (Starting with E)E + D (Using E → E + D)D + D (UsingE → D)D + D * F (Using D → D * F)F + D * F (Using D → F)id + D * F (Using F → id)id + D * id (Using F → id)id + D * id * F (Using D → F)id + id * F (Using F → id)id + id * id (Using F → id)Hence, the string id + id * id can be generated by applying the productions of the given grammar starting with the non-terminal symbol E.。
编译原理第四章答案

“编译技术”第四章作业4-1 已知文法G[C]:C→iEtS|iEtSeSE→ a|bS→ a+b|a*b(1)提取左公共因子;(2)计算修改后文法的每个非终结符的FIRST集和FOLLOW集;(3)给出递归下降分析程序。
(3)递归下降分析程序void match(token t){if(lookahead=='t')lookahead = nexttoken;else error;}void C(){if(lookahead=='i'){match('i');E();if(lookahead=='t'){match('t');S();Cprime();}else error;}else error;}void Cprime(){if(lookahead=='e'){match('e');S();}}void E(){if(lookahead=='a')match('a');else if(lookahead=='b')match('b');else error;}void S(){if(lookahead=='a'){match('a');Sprime();}}void Sprime(){if (lookahead=='+'){match('+');if(lookahed=='b'){match('b');}else error();}else if(lookahead=='*'){match('*');if(lookahead=='b'){match('b');}else error();}}4-2 已知文法G[Z]:Z→Az|bA→ Za|a(1)删除左递归;(2)计算修改后文法的每个非终结符的FIRST集和FOLLOW集;(3)给出递归下降分析程序。
编译原理第四章 习题解答
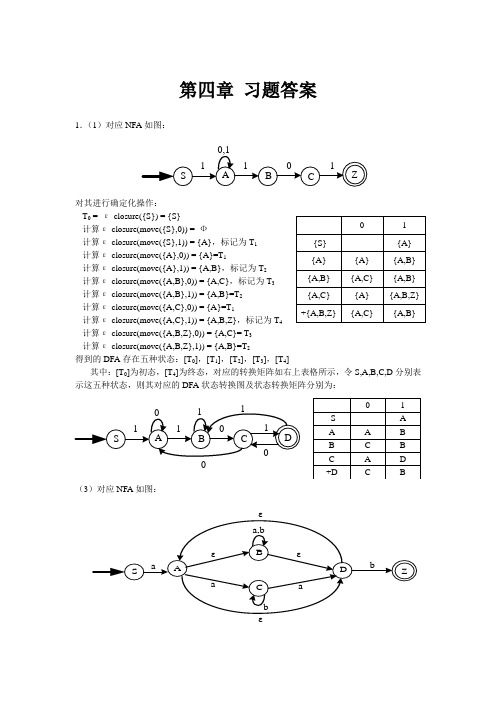
第四章 习题答案1.(1)对应NFA 如图:对其进行确定化操作:T 0 = ε-closure({S}) = {S}计算ε-closure(move({S},0)) = Ф计算ε-closure(move({S},1)) = {A},标记为T 1 计算ε-closure(move({A},0)) = {A}=T 1计算ε-closure(move({A},1)) = {A,B},标记为T 2计算ε-closure(move({A,B},0)) = {A,C},标记为T 3计算ε-closure(move({A,B},1)) = {A,B}=T 2 计算ε-closure(move({A,C},0)) = {A}=T 1计算ε-closure(move({A,C},1)) = {A,B,Z},标记为T 4 计算ε-closure(move({A,B,Z},0)) = {A,C}= T 3 计算ε-closure(move({A,B,Z},1)) = {A,B}=T 2得到的DFA 存在五种状态:[T 0],[T 1],[T 2],[T 3],[T 4]其中:[T 0]为初态,[T 4]为终态,对应的转换矩阵如右上表格所示,令S,A,B,C,D 分别表示这五种状态,则其对应的DFA 状态转换图及状态转换矩阵分别为:(3)对应NFA 如图:εT 0 = ε-closure({S}) = {S}计算ε-closure(move({S},a)) = {A,B,D},标记为T 1 计算ε-closure(move({S},b)) = Ф计算ε-closure(move({A,B,D},a)) = {A,B,C,D},标记为 计算ε-closure(move({A,B,D},b)) = {A,B,D,Z},标记为T 3 计算ε-closure(move({A,B,C,D},a)) = {A,B,C,D}= T 2计算ε-closure(move({A,B,C,D},b)) = {A,B,C,D,Z},标记为T 4 计算ε-closure(move({A,B,D,Z},a)) = {A,B,C,D}= T 2 计算ε-closure(move({A,B,D,Z},b)) = {A,B,D,Z}= T 3 计算ε-closure(move({A,B,C,D,Z},a)) = {A,B,C,D}= T 2 计算ε-closure(move({A,B,C,D,Z},b)) = {A,B,C,D,Z}= T 4 得到的DFA 存在五种状态:[T 0],[T 1],[T 2],[T 3],[T 4]其中:[T 0]为初态,[T 3],[T 4]为终态,对应的转换矩阵如左下表格所示,令S,A,B,C,D 分别2.根据题意,可以得其NFA 状态转换图如下图所示:T 0 = ε-closure({x}) = {x} 计算ε-closure(move({x},0)) = {z},标记为T 1 计算ε-closure(move({x},1)) = {x}=T 0 计算ε-closure(move({z},0)) = {x, z},标记为T 2 计算ε-closure(move({z},1)) = {y},标记为T 3 计算ε-closure(move({x, z},0)) = {x, z}= T 2 计算ε-closure(move({x, z},1)) = {x, y},标记为T 4 计算ε-closure(move({y},0)) = {x, y}= T 4 计算ε-closure(move({y},1)) = Ф计算ε-closure(move({x, y},0)) = {x, y, z},标记为T 5 计算ε-closure(move({x, y},1)) = {x}= T 0计算ε-closure(move({x, y, z},0)) = {x, y, z}= T 5 计算ε-closure(move({x, y, z},1)) = {x, y}= T 4得到的DFA 存在六种状态:[T 0],[T 1],[T 2],[T 3],[T 4],[T 5]其中:[T 0]为初态,[T 1],[T 2],[T 5]为终态,对应的转换矩阵如右上表格所示,令A,B,C,D,E,F 分别表示这六种状态,则其对应的DFA 状态转换图及状态转换矩阵分别为:3.状态转换图如图所示:对其进行确定化操作:T 0 = ε-closure({S}) = {S}计算ε-closure(move({S},0)) = {V ,Q},标记为T 1 计算ε-closure(move({S},1)) = {Q,U},标记为T 2 计算ε-closure(move({V ,Q},0)) = {V,Z},标记为T 3 计算ε-closure(move({V ,Q},1)) = {Q,U}= T 2计算ε-closure(move({Q,U},0)) = {V},标记为T 4计算ε-closure(move({Q,U},1)) = {Q,U,Z},标记为T 5 计算ε-closure(move({V,Z},0)) = {Z}= T 3 计算ε-closure(move({V,Z},1)) = {Z}= T 3计算ε-closure(move({V},0)) = {Z}=T 4 计算ε-closure(move({V},1)) = Ф计算ε-closure(move({Q,U,Z},0)) = {V ,Z},标记为T 6 计算ε-closure(move({Q,U,Z},1)) = {Q,U,Z}= T 5 计算ε-closure(move({Z},0)) = {Z}= T 6 计算ε-closure(move({Z},1)) = {Z}= T 3得到的DFA 存在七种状态:[T 0],[T 1],[T 2],[T 3],[T 4],[T 5],[T 5] 其中:[T 0]为初态,[T 3],[T 5],[T 6]为终态,对应的转换矩阵如右上表格所示,令A,B,C,D,E,F,G 分别表示这七种状态,则其对应的DFA 状态转换图及状态转换矩阵分别为:4.(a )对其进行确定化操作: T 0 = ε-closure({0}) = {0}计算ε-closure(move({0},a)) = {0,1},标记为T 1计算ε-closure(move({0},b)) = {1},标记为T 2计算ε-closure(move({0,1},a)) = {0,1}=T 1计算ε-closure(move({0,1},b)) = {1}= T 2计算ε-closure(move({1},a)) = {0}=T 0 计算ε-closure(move({1},b)) =Ф得到的DFA 存在三种状态:[T 0],[T1],[T 2]其中,[T 0]为初态,[T 0],[T 1]为终态,对应的转换矩阵如右上表格所示,令A,B,C 分别表示这三种状态,则其对应的DFA 状态转换图及状态转换矩阵如下:对其进行最小化操作:该DFA 无多余状态,进行初始划分,得终态组{A,B},非终态组{C}对终态组{A,B}进行审查,输入符号a 后,状态A,B 均转换成状态B ;输入符号b 后,状态A,B 均转换成状态C ,由此可知,状态A,B 等价,不能再分。
编译原理 龙书答案

第四章部分习题解答Aho:《编译原理技术与工具》书中习题(Aho)4.1 考虑文法S →( L ) | aL →L, S | Sa)列出终结符、非终结符和开始符号解:终结符:(、)、a、,非终结符:S、L开始符号:Sb)给出下列句子的语法树i)(a, a)ii)(a, (a, a))iii)(a, ((a, a), (a, a)))c)构造b)中句子的最左推导i)S⇒(L)⇒(L, S) ⇒(S, S) ⇒(a, S) ⇒(a, a)ii)S⇒(L)⇒(L, S) ⇒(S, S) ⇒(a, S) ⇒(a, (L)) ⇒(a, (L, S)) ⇒(a, (S, S)) ⇒(a, (a, S) ⇒(a, (a, a))iii)S⇒(L)⇒(L, S) ⇒(S, S) ⇒(a, S) ⇒(a, (L)) ⇒(a, (L, S)) ⇒(a, (S, S)) ⇒(a, ((L), S)) ⇒(a, ((L, S), S)) ⇒(a, ((S, S), S)) ⇒(a, ((a, S), S)) ⇒(a, ((a, a), S)) ⇒(a, ((a, a), (L)))⇒(a, ((a, a), (L, S))) ⇒(a, ((a, a), (S, S))) ⇒(a, ((a, a), (a, S))) ⇒(a, ((a, a), (a, a))) d)构造b)中句子的最右推导i)S⇒(L)⇒(L, S) ⇒(L, a) ⇒(S, a) ⇒(a, a)ii)S⇒(L)⇒(L, S) ⇒ (L, (L)) ⇒(L, (L, S)) ⇒(L, (L, a)) ⇒(L, (S, a)) ⇒(L, (a, a)) ⇒(S, (a, a)) ⇒(a, (a, a))iii)S⇒(L)⇒(L, S) ⇒(L, (L)) ⇒(L, (L, S)) ⇒(L, (L, (L))) ⇒(L, (L, (L, S))) ⇒(L, (L, (L,a))) ⇒(L, (L, (S, a))) ⇒(L, (L, (a, a))) ⇒(L, (S, (a, a))) ⇒(L, ((L), (a, a))) ⇒(L, ((L,S), (a, a))) ⇒(L, ((L, a), (a, a))) ⇒(L, ((S, a), (a, a))) ⇒(L, ((a, a), (S, S))) ⇒(S, ((a,a), (a, a))) ⇒(a, ((a, a), (a, a)))e)该文法产生的语言是什么解:设该文法产生语言(符号串集合)L,则L = { (A1, A2, …, A n) | n是任意正整数,A i=a,或A i∈L,i是1~n之间的整数}(Aho)4.2考虑文法S→aSbS | bSaS | εa)为句子构造两个不同的最左推导,以证明它是二义性的S⇒aSbS⇒abS⇒abaSbS⇒ababS⇒ababS⇒aSbS⇒abSaSbS⇒abaSbS⇒ababS⇒ababb)构造abab对应的最右推导S⇒aSbS⇒aSbaSbS⇒aSbaSb⇒aSbab⇒ababS⇒aSbS⇒aSb⇒abSaSb⇒abSab⇒ababc)构造abab对应语法树d)该文法产生什么样的语言?解:生成的语言:a、b个数相等的a、b串的集合(Aho)4.3 考虑文法bexpr→bexpr or bterm | btermbterm→bterm and bfactor | bfactorbfactor→not bfactor | ( bexpr ) | true | falsea)试为句子not ( true or false)构造分析树解:b)试证明该文法产生所有布尔表达式证明:一、首先证明文法产生的所有符号串都是布尔表达式变换命题形式——以bexpr、bterm、bfactor开始的推导得到的所有符号串都是布尔表达式最短的推导过程得到true、false,显然成立假定对步数小于n的推导命题都成立考虑步数等于n 的推导,其开始推导步骤必为以下情况之一bexpr⇒bexpr or btermbexpr⇒btermbterm⇒bterm and bfactorbexpr⇒bfactorbfactor⇒not bfactorbfactor⇒ ( bexpr )而后继推导的步数显然<n,因此由归纳假设,第二步句型中的NT推导出的串均为布尔表达式,这些布尔表达式经过or、and、not运算或加括号,得到的仍是布尔表达式因此命题一得证。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
第四章
习题4.2.1:考虑上下文无关文法: S->S S +|S S *|a 以及串aa + a*
(1)给出这个串的一个最左推导
S -> S S *
-> S S + S *
-> a S + S *
-> a a + S *
-> aa + a*
(3)给出这个串的一棵语法分析树
习题4.3.1:下面是一个只包含符号a和b的正则表达式的文法。
它使用+替代表示并运算的符号|,以避免和文法中作为元符号使用的竖线相混淆:
rexpr→ rexpr + rterm | rterm
rterm→rterm rfactor | rfactor
rfactor→ rfactor * | rprimary
rprimary→a | b
1)对这个文法提取公因子
2)提取公因子的变换使这个文法适用于自顶向下的语法分析技术吗?
3)提取公因子之后,原文法中消除左递归
4)得到的文法适用于自顶向下的语法分析吗?
解
1)提取左公因子之后的文法变为
rexpr→ rexpr + rterm | rterm
rterm→rterm rfactor | rfactor
rfactor→ rfactor * | rprimary
rprimary→a | b
2)不可以,文法中存在左递归,而自顶向下技术不适合左递归文法
3)消除左递归后的文法
rexpr -> rterm rexpr’
rexpr’-> + rterm rexpr’|ε
rterm-> rfactor rterm’
rterm’-> rfactor rterm’|ε
rfactor-> rprimay rfactor’
rfactor’-> *rfactor’|ε
rprimary-> a | b
4)该文法无左递归,适合于自顶向下的语法分析
习题4.4.1:为下面的每一个文法设计一个预测分析器,并给出预测分析表。
可能要先对文法进行提取左公因子或消除左递归
(3)S->S(S)S|ε
(5)S->(L)|a L->L,S|S
解
(3)
①消除该文法的左递归后得到文法
S->S’
S’->(S)SS’|ε
②计算FIRST和FOLLOW集合
FIRST(S)={(,ε} FOLLOW(S)={),$}
FIRST(S’)={(,ε} FOLLOW(S’)={),$}
③
(5)
①消除该文法的左递归得到文法
S->(L)|a
L->SL’
L’->,SL’|ε
②计算FIRST与FOLLOW集合
FIRST(S)={(,a} FOLLOW(S)={ ),, ,$}
FIRST(L)={(,a} FOLLOW(L)={ ) }
FIRST(L’)={,,ε} FOLLOW(L’)={ ) }
③
习题4.4.4 计算练习4.2.2的文法的FIRST和FOLLOW集合3)S→S(S)S|ε
5)S→(L)|a,L→L,S|S
解:
3)FIRST(S)={ ε,( } FOLLOW(S)={ (,),$ }
5)FIRST(S)={ (,a } FOLLOW(S)={ ),,,$ }
FIRST(L)={ (,a } FOLLOW(L)={ ),, }
习题4.6.2为练习4.2.1中的增广文法构造SLR项集,计算这些项集的GOTO函数,给出这个文法的语法分析表。
这个文法是SLR文法吗?
S→SS+|SS*|a
解:
①构造该文法的增广文法如下
S’->S
S->SS+
S->SS*
S->a
②构造该文法的LR(0)项集如下
③GOTO函数如下
GOTO(I0,S)=I1 GOTO(I0,a)=I2
GOTO(I1,S)=I3 GOTO(I1,a)=I2 GOTO(I1,$)=acc
GOTO(I3,S)=I3 GOTO(I3,+)=I4 GOTO(I3,*)=I5 GOTO(I3,a)=I2
④构造该文法的语法分析表
注:FOLLOW(S’)=FOLLOW(S)={+,*,a,$}
这个文法是SLR文法,因为语法分析表中没有重复的条目
习题4.6.6说明下面文法
S→SA|A
A→a
是SLR(1)的,而不是LL(1)的。
证明:
1)可以求得FIRST(SA)=FIRST(A)={a},故该文法不是LL(1)文法
2)构造该文法的增广文法的语法分析表如下
①构造增广文法
S’->S
S->SA
S->A
A->a
②构造LR(0)项集族
③GOTO函数如下
GOTO(I0,S)=I1 GOTO(I0,A)=I2 GOTO(I0,a)=I3
GOTO(I1,A)=I4 GOTO(I1,a)=I3 GOTO(I1,$)=acc
④构建语法分析表如下(FOLLOW(A)=FOLLOW(S)={a,$})
可以看到该语法分析表中没有重复的条目故该文法是SLR(1)文法
习题4.7.4说明下面的文法
S->Aa|bAc|dc|bda
A->d
是LALR(1)的,但不是SLR(1)的
证明:
1、构造该文法的SLR(1)语法分析表
①构造增广文法
S’->S
S->Aa
S->bAc
S->dc
S->bda
A->d
②构造LR(0)项集族
③GOTO函数
GOTO(I0,S)=I1 GOTO(I0,A)=I2 GOTO(I0,b)=I3 GOTO(I0,d)=I4 GOTO(I1,$)=acc GOTO(I2,a)=I5 GOTO(I3,A)=I6 GOTO(I3,d)=I7 GOTO(I4,c)=I8 GOTO(I6,c)=I9 GOTO(I7,a)=I10
④构建SLR语法分析表如下(FOLLOW(A)={a,c})
可以看到在图中存在二义性的条目,故该文法不是SLR(1)文法
2、构造该文法的LALR(1)语法分析表
①构造该增广文法的LR(1)项集族如下
③GOTO函数
GOTO(I0,S)=I1 GOTO(I0,A)=I2 GOTO(I0,b)=I3 GOTO(I0,d)=I4 GOTO(I1,$)=acc GOTO(I2,a)=I5 GOTO(I3,A)=I6 GOTO(I3,d)=I7
GOTO(I4,c)=I8 GOTO(I6,c)=I9 GOTO(I7,a)=I10
可见该分析表中不存在二义性的条目,故该文法是LALR(1)文法
习题4.7.5说明下面的文法
S->Aa|bAc|Bc|bBa
A->d
B->d
是LR(1)的,但不是LALR(1)的
证明:
1、构造该文法的LR(1)语法分析表
①构造该文法的增广文法
S’->S
S->Aa
S->bAc
S->Bc
S->bBa
A->d
B->d
②构造该增广文法的LR(1)项集族如下
②项集合并:没有可以合并的项集
③GOTO 函数
GOTO(I0,S)=I1 GOTO(I0,A)=I2 GOTO(I0,b)=I3 GOTO(I0,B)=I4 GOTO(I0,d)=I5 GOTO(I1,$)=acc GOTO(I2,a)=I6 GOTO(I3,A)=I7 GOTO(I3,B)=I8 GOTO(I3,d)=I9 GOTO(I4,c)=I10 GOTO(I7,c)=I11 GOTO(I8,a)=I12
2、 构造该文法的LALR(1)语法分析表 ①合并LR(1)项集族 I5和I9可以合并为I59。
