美国“高考”SAT考试的数学题

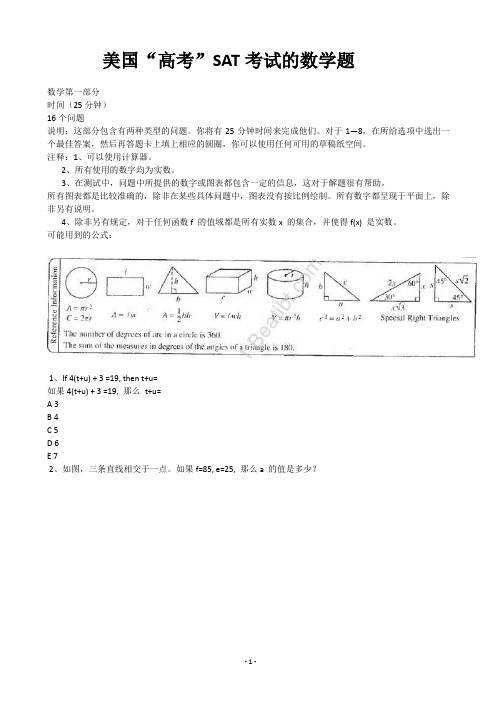
美国“高考”SAT考试的数学题

数学第一部分

时间（25分钟）

16个问题

说明：这部分包含有两种类型的问题。你将有25分钟时间来完成他们。对于1—8，在所给选项中选出一个最佳答案，然后再答题卡上填上相应的圆圈，你可以使用任何可用的草稿纸空间。

注释：1、可以使用计算器。

2、所有使用的数字均为实数。

3、在测试中，问题中所提供的数字或图表都包含一定的信息，这对于解题很有帮助。

所有图表都是比较准确的，除非在某些具体问题中，图表没有按比例绘制。所有数字都呈现于平面上，除非另有说明。

4、除非另有规定，对于任何函数f 的值域都是所有实数x 的集合，并使得f(x) 是实数。

可能用到的公式：

1、If 4(t+u) + 3 =19, then t+u=

如果4(t+u) + 3 =19, 那么t+u=

A 3

B 4

C 5

D 6

E 7

2、如图，三条直线相交于一点。如果f=85, e=25, 那么a 的值是多少？

A 60

B 65

C 70

D 75

E 85

A n/t

B t/n

C 1/nt

D nt

E n²t

4、如果a 是一个奇数，b 是一个偶数，那么选项中哪一个是奇数？

A 3b

B a+3

C 2(a+b)

D a+2b

E 2a+b

5、在平面坐标内，F(-2,1),G(1,4), H(4,1)在以P为圆心的圆上，那么点P的坐标是什么？

A（0,0）

B（1,1）

C（1,2）

D（1，-2）

E（2.5,2.5）

6、如图，如果-3≤x≤6，那么x 有几个值，使得f(x)=2?

A 零

B 一个

C 两个

D 三个

E 三个以上

7、如果t 和t+2 的算术平均值是x, t 和t-2的算术平均值是y，那么x 和y 的算术平均值是多少？

A 1

B 1/2

C t

D t+1/2

E 2t

8、对于任何数x 和y,假设x△y=x²+xy+y²,那么（3△1）△1等于多少？

A 5

B 13

C 27

D 170

E 183

9、摩根的植物在一年之内从42厘米长到57厘米。而琳达的植物年前是59厘米，一年之内她的植物生长的高度是摩根植物长高的两倍，那么，在年底琳达植物的高度是多少？

10、从1990年开始，一个树木繁茂区的松鼠的数量以每三年三倍的速度在增长。如果1999年初这个地区有松鼠5400只，那么在1990年初的时候这个地区有多少松鼠？

11、如图，ΔABC和ΔDCE均是等边三角形，且AE长为25.求这两个等边三角形的周长一共是多少？

少弹珠才能使剩余在坛子中的红色弹珠与黑色弹珠的比例为4：3？

13、已知X=3v ,V=4t ，X=pt如果x≠0，求p的值？

14、如果｜-2x+1｜＜1,找出x的一个可能值？

15、有一个正数，它是一个数的平方根，并且这个数也是40的一个约数，那么这个正数是多少？

16、如下图，矩形ABDF, C和E分别是边BD、DF的中点。那么阴影ACEF部分的面积占了这个矩形的几分之几？

数学第二部分

时间（25分钟）

16个问题

说明：这部分包含有两种类型的问题。你将有25分钟时间来完成他们。对于1—8，在所给选项中选出一个最佳答案，然后再答题卡上填上相应的圆圈，你可以使用任何可用的草稿纸空间。

注释：1、可以使用计算器。

2、所有使用的数字均为实数。

3、在测试中，问题中所提供的数字或图表都包含一定的信息，这对于解题很有帮助。

所有图表都是比较准确的，除非在某些具体问题中，图表没有按比例绘制。所有数字都呈现于平面上，除非另有说明。

4、除非另有规定，对于任何函数f 的值域都是所有实数x 的集合，并使得f(x) 是实数。

1、下列哪一项不是80的因数？

A、5

B、8

C、12

D、16

E、40

2、已知k=3wx,m=(w-1)k.当w=4,x=1时，m的值是多少？

A、0

B、3

C、12

D、24

E、36

3、如图，街道的两边各有5间房子，若给这五间房子涂色，且相邻或正面相对的两间房子不可以涂相同的颜色，如果标有G的房子已涂了灰色，那么剩余7间房子有几间不可以涂成灰色？

4、如果7n×7=7¹²,（7n为7的n次方）那么n 的值是多少？

A、2

C、9

D、15

E、36

5、如图，一家具公司生产桌椅，左图为其在3年中桌椅的不同销售价格；右图为三个仓库所能存放的货物最大容量。根据图表所示价格，在1995年，y仓库所存桌椅的价值最大是多少？

6、如图，图中哪个角最大？

A、a

B、b

C、c

D、d

E、e

7、下列哪个方程式可能是图中函数的方程式？

A、y=x²+2

B、y=(x+2)²

C、y=x²-2

D、y=(x-2)²

E、y=2x²

8、立方体的边所形成的直角一共有多少个？

A、36

B、24

C、20

D、16

E、12

9、如果（p+1）(t-3)=0,并且p是正数，那么t的值是多少？

A、-3

B、-1

C、0

D、1

E、3

10、表中x,y满足下面那个等式？

A、y=100-x²

B、y=100-x

C、y=100-2x