凸函数的性质及其在证明不等式中的应用
函数的凹凸性在不等式证明中的应用

函数的凹凸性在不等式证明中的应用函数的凹凸性是高等数学中的一个重要概念,它描述了函数图像的形状。
具体来说,如果函数的图像在一些区间上是向上凸起的,我们称之为凸函数;如果函数的图像在一些区间上是向下凹陷的,我们称之为凹函数。
在不等式证明中,函数的凹凸性具有很重要的应用。
首先,函数的凹凸性可以帮助我们证明不等式的性质。
假设我们要证明一个不等式,例如a + b ≥ 2√(ab),其中a、b为非负实数。
我们可以考虑定义函数f(x) = x²,则f(x)是一个凸函数。
由凸函数性质可知,对于任意的实数x₁、x₂,有f(λx₁ + (1 - λ)x₂) ≤ λf(x₁) + (1 -λ)f(x₂),其中0 ≤ λ ≤ 1、将x₁ = a,x₂ = b代入上述不等式,可得2ab ≤ a² + b²。
再将a² + b²除以2,即可得到a + b ≥ 2√(ab)。
因此,通过证明函数的凹凸性,我们可以得到不等式的性质。
其次,函数的凹凸性还可以帮助我们求解优化问题。
假设我们要在非负实数集合中找到满足一些条件的最大值或最小值。
我们可以先通过求导得到函数的极值点,然后通过函数的凹凸性判断这个极值点是最大值还是最小值。
具体来说,如果函数是凸函数,那么极值点就是最小值;如果函数是凹函数,那么极值点就是最大值。
通过函数的凹凸性,我们可以在优化问题中确定最优解。
此外,函数的凹凸性还可以帮助我们证明不等式的反面。
例如,我们要证明a + b ≥ 2√(ab),其中a、b为非负实数。
假设我们采用反证法,假设不等式不成立,即a + b < 2√(ab)。
我们可以定义函数f(x) = x - 2√(x),其中x为非负实数。
我们可以证明函数f(x)是一个凹函数,然后通过证明f(a) + f(b) < 0,来推出假设的不等式不成立。
通过函数的凹凸性,我们可以证明不等式的反面。
总的来说,函数的凹凸性在不等式证明中具有重要的应用。
凸函数的性质与应用

凸函数的性质与应用数学与统计学院、数学与应用数学、0701班,湖北,黄石,4350021.引言凸函数是数学分析中的一个重要概念,它涉及了许多数学命题的讨论证明和应用,而且在现代优化学、运筹学、管理学、和工程测绘学等多个学科有着重要的意义和很好的应用.关于凸函数,虽然很多书籍都做了相应的介绍,但多是从不同的角度出发来进行不同的定义和应用.在高等数学中,利用导数讨论函数的性态时,经常遇到一类特殊函数—凸函数,由于凸函数具有一些特殊性质,利用这些性质可非常简单地证明一些初等不等式、函数不等式和积分不等式. 凸函数是一类重要的函数,在不等式的研究中尤为重要.本文通过凸函数的定义、性质的描述,主要研究其在不等式证明中的应用,举例说明解题思路与证明方法,并且证明了几个常见的重要不等式及高次函数的凸性猜想. 函数的凸性是函数在区间上变化的整体性态,把握函数在区间上的整体性态,不仅可以更加科学、准确地描绘函数的图象,而且有助于对函数的定性分析.2. 凸函数的有关概念2.1凸函数的定义、定理及其几何意义定义 若函数()f x 对于区间(),a b 内的任意12,x x 以及()0,1,λ∈恒有1212[(1)]()(1)()f x x f x f x λλλλ+-≤+-,则称()f x 为区间(),a b 上的凸函数.其几何意义为:凸函数曲线()y f x =上任意两点()()11,,x f x ()()22,x f x 间的割线总在曲线之上.定理1 若函数()f x 在区间(),a b 内连续,对于区间(),a b 内的任意12,x x 恒有12121[][()()]22x x f f x f x +≤+, 则称()f x 为区间(),a b 上的凸函数.其几何意义为:凸函数曲线()y f x =上任意两点()()11,,x f x ()()22,x f x 间割线的中点总在曲线上.定理2 若函数()f x 在区间(),a b 内可微,且对于区间(),a b 内的任意x 及0x ,恒有00()()()f x f x f x x '≥+-,则称()f x 为区间(),a b 上的凸函数.其几何意义为:凸函数曲线()y f x =上任一点处的切线,总在曲线之下. 注 若将定义1,2,3中的≤“”改为<“”则称()f x 为(),a b 上的严格凸函数. 2.2 凸函数定义与定理之间的等价性条件2.2.1 定义1与定理1的等价性证 定义1⇒定理1:显然,只要取12λ=即可由定义1推得定理1.定理1⇒定义1:我们首先推证()f x 对于任意的12,x x (),a b ∈及有理数()0,1λ∈,不等式1212[(1)]()(1)()f x x f x f x λλλλ+-≤+-,成立.事实上,对于此有理数λ,总可表示为有穷二进位小数,即121121122220.2n n n nn na a a a a a a ---++= , 其中0,1(1,2,,1);1i n a i n a ==-= 或由于1λ-也是有理数,故也可以表示为有穷的二进位小数,即1λ-=121121122220.2n n n nn nb b b b b b b ---++= , 其中()1,1,2,,1;i i b a i n =-=- 1,n b =这是因为()11λλ+-=的缘故, 因此111212[]()()i i f a x b x a f x b f x +≤+(1,2,,1)i n =- ,所以12[(1)]f x x λλ+-12112112112112222222[]22n n n n n n n nn na a a ab b b b f x x ------++++=+ 21212121111212112222()(22[]2n n n n n nn n a a a b b b a x b x x x f ------+++++= 2121212111121211222211[()]()2222n n n nn n n n a a a b b b f a x b x f x x ------++≤+++ 2121212111121211222211[()()]()2222n n n n n n n n a a a b b b a f x b f x f x x ------++≤+++ 121112212221111[()()][()()]()2222n n n a x b x a f x b f x a f x b f x f -+≤++++ 11122122122111[()()][()()][()()]222n n n a f x b f x a f x b f x a f x b f x ≤+++++12112112112112222222()()22n n n n n n n n n na a a ab b b b f x f x ------++++=+ 12()(1)().f x f x λλ=+-下面再推证()f x 对λ为无理数时定义1也成立.事实上,对任意无里数()0,1,λ∈{}(0,1),n λ⊂存在有理数列12(),(1)n n n n x x λλλλ→→∞+-→所以,12(1)()x x n λλ+-→∞,由于()f x 在(),a b 内连续,所以1212121212[(1)][lim (1)]lim [(1)]lim[()(1)()]()(1)()n n x n n n n x x f x x f x x f x x f x f x f x f x λλλλλλλλλλ→∞→∞→∞+-=+-=+-≤+-=+-综上即知,定义1与定理1等价.2.2.2 定义1与定理2的等价条件证 定义1⇒定理2:对(),a b 内任意的0x 及x ,若0,x x <则取0h >,使00,x x h x <+<由推论1得0000()()()()].f x h f x f x f x h x x +-+≤-上式中令0,h →由于()f x 可微,所以有0()f x '00()(),f x f x x x +≤-即00()()()f x f x f x x '≥+-.若0,x x <则取0h >,使00,,x x x x h x <<+<同理可证.2.2.3 定理2与定义1的等价条件对于区间(),a b 内的任意12,x x (不妨设12x x <)以及()0,1,λ∈令()121x x x λλ=+-,则12,x x x << ()()1121,x x x x λ-=-- 2x x -= ()()211,x x λ--由泰勒(Taylor)公式,我们有111222()()()()()()()()f x f x f x x f x f x f x x θθ''=+-=+-及其中1122x x x θθ<<<<,于是12()(1)()f x f x λλ+-12[(1)]f x x λλ=+-+2121(1)()[()()]x x f f λλθθ''---.再由单调性知21()()f f θθ''≥,所以12()(1)()f x f x λλ+-≥ 12[(1)]f x x λλ+-,即12[(1)]f x x λλ+-≤12()(1)()f x f x λλ+-.所以在一定条件下,定义1与定理3等价.3. 凸函数的有关结论 3.1 凸函数的运算性质性质1 若()f x 为区间I 上的凸函数, k 为非负实数,则()kf x 也为区间I 上的凸函数.性质2 若()(),f x g x 均为区间I 上的凸函数,则()f x + ()g x 也为区间I 上的凸函数.推论 若()(),f x g x 均为区间I 上的凸函数,12,k k 为非负实数,则()()12f x k g x +k 也为区间I 上的凸函数.性质3 若()f x 为区间I 上的凸函数,()g x 为J 上的凸增函数,且()f I J ⊂,则g f ⋅为区间I 上的凸函数.性质4 若()(),f x g x 均为区间I 上的凸函数,则()F x =()(){}max ,f x g x 也是区间I 上的凸函数.上述性质很容易证明,故在此省略.3.2 凸函数的其他性质引理 f 为I 上的凸函数的充要条件是:对于I 上的任意三点12x x x <<,总有32212132()()()()f x f x f x f x x x x x +-≤--. ()1证 [必要性]记3231,x x x x λ-=-则213(1).x x x λλ=+- 由f 的凸性知道()21313[(1)]()(1)()f x f x x f x f x λλλλ=+-≤+-=3221133131()()x x x xf x f x x x x x --+--.从而有()()312321213()()()()x x f x x x f x x x f x -≤-+-,即()()()322212321213()()()()()x x f x x x f x x x f x x x f x -+-≤-+-.整理后即得()1式.[充分性]在I 上任取两点1313,,(),x x x x <在[13,x x ]上任取一点213(1)x x x λλ=+- ()0,1,λ∈即3231.x x x x λ-=-由必要性的推导逆过程,即可证明 1313[(1)]()(1)()f x x f x f x λλλλ+-≤+-.故f 为I 上的凸函数.同理可证,f 为I 上的凸函数的充要条件是:对于I 上的任意三点12,x x x <<总有313221213132()()()()()()]]f x f x f x f x f x f x x x x x x x -+-≤≤---.性质1 设f 为区间I 上的严格凸函数,若有0x 是()f x 的极小值点,则0x 是()f x 在I 上唯一的极小值点.证明 若()f x 有异于0x 的另一极小值点1x I ∈ ,不妨设()()10f x f x ≤ 由于()f x 是在I 上的严格凸函数, 故对于任意的()0,1λ∈,都有()01010[(1)]()(1)()f x x f x f x f x λλλλ+-<+-≤.于是,任意的0δ>,1,只要充分接近时总有()0010(1),x x x U x λλδ=+-∈.但是,()0()f x f x ≤,这与1x 是()f x 的极小值点的条件矛盾,从而0x 是()f x 在I 上唯一的极小值点.性质2 设()f x 为(),a b 内的凸函数,有()f x 在I 的任一内闭区间()(),,a b αβ<上满足Lipschitz 条件.证明 要证明()f x 在(),αβ上满足Lipschitz 条件,即要证明:0,L ∃>使得()12,,x x αβ∀∈有1212()()f x f x L x x -≤-. ()2()()()(),,,,,,a b h h a b αβαβ⊂-+⊂因为,故可取充分小使得因此,()12,,x x αβ∀∈,12,x x <32x x h =+取,根据定义有32212132()()()()f x f x f x f x M mx x x x h+--≤≤--,(其中,M m 分别表示()f x 在(),h h αβ-+的上、下界)从而2121()()M mf x f x x x h--≤-, ()3 若1232,,x x x x h >=-可取由定义有32211223()()()()f x f x f x f x x x x x --≤--,从而32211223()()()()f x f x f x f x M m x x x x h---≤≤--.由此也可推出()3式.若12x x =,则()2显然成立.这就证明了()3式显然对于一切()12,,x x αβ∈都成立,因此()3式当12,x x 互换位置也应成立,故有2121()()M mf x f x x x h--≤-. 令M mL h-=,则原命题成立.性质3 设()f x 是(),a b 上的凸函数,则()f x 在(),a b 上处处存在左、右导数,且左导数小于、等于右导数.证明 ()()()00,,,x a b U x a b δ∀∈∃⊂.记()()00()(),,f x f x F x x a b x x +=∈-,()121200,x x x x x x δ<∈-任意且,,,有引理得()()1020121020()()()()f x f x f x f x F x F x x x x x ++=≤=--.()F x 在()00x x δ-,上单调递增;再在0x 右方任取一定点()00,x x λλδ∈+,,由引理得: ()()()12F F F x x λ≤≤所以()F x 在()00x x δ-,上单调递增且有上界, 故由单调有界原理: 极限()0lim x x F x -→存在,即0()f x +'存在; 任意102x x x <<由定义3有()()1020121020()()()()f x f x f x f x F x F x x x x x ++=≤=--.令1020,x x x x -+→→,则()f x 在0x 的左导数小于等于()f x 在0x 的右导数.性质4 设()f x 为(),a b 内可导凸函数,证明()0,x a b ∈ 为()f x 的极小值的充要条件是0()0f x '=.证明 [必要性]已知函数()f x 在0x 可导,且取得极小值,则0()0f x '=(极值的必要条件).[充分性] (),x a b ∀∈,0,x x ≠有00()()().f x f x x x ≥+-因为0()0f x '=,故(),,x a b ∀∈都有0()(),f x f x ≥所以0x 为()f x 的极小值点.定理1 设f 为区间I 上的可导函数,则下列论断互相等价;1) f 为I 上的凸函数, 2) f '为I 上的增函数, 3) 对I 上的任意两点12,,x x 有()21121()()()f x f x f x x x '≥+-. ()*证明 1)2)→ 任取I 上的两点1212,x x x x <()及充分小的正数,h 由于1122,x h x x x h -<<<+根据的凸性及引理有11212212()()()()()()f x f x h f x f x f x h f x h x x h---+-≤≤-.有f 是可导函数,令0h +→时可得211212()()()()f x f x f x f x x x -''≤≤-.所以f '为I 上的递增函数.2)3)→ 在以1212,()x x x x <为端点的区间上,应用拉格朗日中值定理和f '递增条件,有()()2121121()()()()f x f x f x x f x x x ξ''-=-≥-,移项后即得()*式成立,且当12x x >仍可得到相同结论3)1)→ 设以12,x x 为I 上的任意两点,312(1)x x x λλ=+-,由3)并利用131223211)()x x x x x x x x λλ-=---=-与(),()()133133312()()()()(1)()f x f x f x x x f x f x x x λ''≥+-=+--,()233233321()()()()()f x f x f x x x f x f x x x λ''≥+-=+-(),分别用λ和1λ-乘上列两式并相加,便得()()12312(1)()()(1)f x f x f x f x x λλλλ+-≥=+-,从而为I 上的凸函数.推论1 设()f x 为区间I 上的二阶可导函数,则()f x 为凸函数.()0,f x x I ''⇔≥∈.推论2 设()f x 为区间I 上的可微凸函数,则有0x I ∈是()f x 的极小值点.()00.f x ''⇔=定理2 设()f x 在(),a b 上连续,则()f x 是(),a b 上的凸函数的充要条件是:对任意含于(),a b 的闭区间[],,x h x h -+都有1()()2hhf x f x t dt h -≤+⎰. 证明 必要性:()()()()1,2t h f x f x t f x t ∀≤≤-++,故 ()()()()12[]2hhhhhf x f x t f x t f x t dt --≤-++≤+⎰⎰.充分性:假定存在12,x x <使()()1212122x x f f x f x +⎛⎫>+⎡⎤ ⎪⎣⎦⎝⎭ 作辅助函数()()()()11,x f x k x x f x ϕ=---其中2121()()f x f x k x x +=-则120,2x x ϕ+⎛⎫> ⎪⎝⎭因此[]()()[][]12012,max 0,0,,,,x x x x h x h x h x x ϕϕ=>=-+⊂取()()000t h x x t ϕϕ≤-+≥当时,且不恒为0,因此()()002hhh x xt dt ϕϕ->+⎰.再由()x ϕ的定义推出: ()002()hhf x t hf x dt -+>⎰这与条件矛盾, 故定理2得证.定理3 若()f x 为(),a b 内的凸函数,(),,i x a b ∈ 1,2,,,i n = 则()111.ni ni i i x f f x n n ==⎛⎫⎪ ⎪≤ ⎪ ⎪⎝⎭∑∑ 证明 对12,2n x ==不等式是显然的,设对1n -不等式成立. 因为1212111,1n n n x x x x x x n x n n n n-++++++-=⋅+-这里()()1211,,,,,1n n x x x n a b x a b n n λ-+++-=∈∈- 由题得()()111111.1nn i i n i i n i i x x n f f f x f x n n n nn ===⎛⎫⎛⎫ ⎪ ⎪- ⎪⎪≤+=- ⎪ ⎪ ⎪ ⎪⎝⎭⎝⎭∑∑∑ 4.凸函数的一些应用4.1应用凸函数性质证明不等式在初等数学及数学分析的课程中,对于不等式的证明是一个重要内容.有时利用凸 函数的理论,证明一些不等式,将会更加简单.下面用例题加以说明.例1 求证:对任意实数,,a b 有()21.2a ba bee e +≤+ 证明 设()()(),0,,x f x e f x x ''=≥∈-∞+∞则故()xf x e =(),-∞+∞为上的凸函数.从而对121,,2x a x b λ===有定义 12121[][()()]22x x f f x f x +≤+. 即得()212a ba bee e +≤+. 注:该题构造函数,运用凸函数的定义很容易就导出.例2 设01,01,x a <<<<则有()()1111.aax x x -+-<-证明 设()()()()11101aaf x x x x -=+-<<.那么()()()()()()111111,aaaa f x a x x x ax ---'=-+-++-()()()()()()1111111aaa a f x a a x x a a x x ----''=--+---+()()()()1121111aaa a a a x x a a x x ------+--+()()()()()()12112111111aa a a a a a x x x x x x x x -----⎡⎤=--+-++++-+⎣⎦()()()()()()1212111111.a a aa a a x x a a x x ------=--+-=-+-于是 ,当01,01x a <<<<时,()0,f x ''>由严格凸函数的定义,其中12,1,0,x x x λ===得()()()()()110110,f x f x x x f x f =⋅+-⋅<⋅+-⋅⎡⎤⎣⎦即()()1111.aax x x -+-<-注:该题运用了定理1及推论1的结论.例3 在ABC 中,证明sin sin sin 2A B C ++()()()()sin ,0,,sin 0,0,f x x x f x x x ππ''=-∈=>∈证明 令由应用2得()()()33f A f B f C A B C F ++++⎛⎫≥ ⎪⎝⎭,即sin sin sin sin3A B CA B C ++++≤s i n ,3π≤=所以sinA+sinB+sinC 2注:该题运用了定理3的结论.例4设12n a a a 、、均为正数,且121n a a a +++= .求证: ()2222212121111.n n n a a a a a a n +⎛⎫⎛⎫⎛⎫++++++≥⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭证 因为()2,f x x =()()()22,20,f x x f x f x x ''==>=由于得是凸函数,有凸函数的性质,有22212122121221211111111111.n n n n n a a a a a a a a a a a a n n n a a a ⎛⎫⎛⎫⎛⎫+++++ ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭⎛⎫++++++ ⎪⎪≥⎪ ⎪⎝⎭⎛⎫=++++ ⎪⎝⎭()4由柯西不等式:222111n n n i i i i i i i a b a b ===⎛⎫⎛⎫⎛⎫⋅≥ ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭∑∑∑得1212111111()1n n a a a a a a ⎛⎫+++=+++⋅ ⎪⎝⎭()12122111(),n n a a a a a a n =++++++≥212111()nn a a a ∴+++≥ ,由()4即得 ()2222212121111n n n a a a a a a n+⎛⎫⎛⎫⎛⎫+++++≥⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭ .4.2关于凸函数的某些猜想猜想1 三次函数不是(),-∞+∞上的凸函数. 证 设()3232103,0.x x a x a x a a f a +++≠= 显然,()f x 在(),-∞+∞上可导,且()232123x x a x a f a ++'=,因为30,a ≠故()f x '在(),-∞+∞上不单调,所以不是凸函数.猜想2 试给出四次的函数在定义域上是凸函数的一个充分条件. 设()432432104,0,x x x a x a x a a f a a ++++≠=因为四次的在定义域上二次同样可导,且()324321432x x x a x a f a a +++'=, ()24321262x x x a f a a ++''=.根据3..1的推论1可知,下式()423420.64120a a a a >⎧⎪⎨-⋅⋅≤⎪⎩ 则该四次函数在(),-∞+∞是凸函数. 化简得① 423420.380a a a a >⎧⎨-⋅≤⎩ ② 423420.380a a a a <⎧⎨-⋅≤⎩ 则该四次函数在(),-∞+∞不是凸函数.③ 423420.380a a a a >⎧⎨-⋅>⎩设()24321262x x x a f a a ++''=与x 轴的两交点分别是()1212,,x x x x <则()x f 在()()12,,,x x -∞+∞内分别为凸函数,在()12,x x 内不是凸函数.④ 423420.380a a a a <⎧⎨-⋅>⎩ 同理设()x f ''与x 轴的两交点分别是()1212,,x x x x <则()x f 在()12,x x 内为凸函数,其他区间不是凸函数.猜想3 5次函数在实数范围内是否有为凸函数的?设5次函数的表达式为()54325432105,0,x x x x a x a x a a f a a a +++++≠= 显然该是在实数范围内二次可导.()432543215432,x x x x a x a f a a a ++++'= ()325432201262.x x x x a f a a a +++''=现在需要找出二次导数在实数范围内是否恒大于等于0. 我们设()()325432201262,x f x x x x a g a a a ''=+++=()2154360246.x x x g a a a =++'下面分情况讨论:()524530,2446060a a a a >⎧⎪⎨-⋅⋅≤⎪⎩ 即()0x g ≥'在R 上恒成立.则()x g 在R 上单调递增,此时5a 为某一定值,但是总,x R ∃∈使得()0,x g <即x R ∃∈使()0f x ''<成立.同四次的理一样,其他3种情况更不可能为凸函数. 所以五次函数在R 上不是凸函数.以此类推,高次函数()11100,,n n n n n f x a x a x a x a a --=+++≠5n 时,该函数在实数范围内不是凸函数.5.小结本文通过凸函数的定义、性质的描述,主要研究其在不等式证明中的应用,举例说明解题思路与证明方法,并且证明了几个常见的重要不等式及高次函数的凸性猜想.函数的凸性是函数在区间上变化的整体性态,把握函数在区间上的整体性态,不仅可以更加科学、准确地描绘函数的图象,而且有助于对函数的定性分析.致谢经过半年的忙碌和工作,本次毕业论文已经接近尾声,在这里首先要感谢我的指导老师柴国庆教授.柴老师平日里工作繁多,但在我做毕业论文的每个阶段,从初次选题到查阅资料,论文初稿的确定和修改,中期检查,后期详细设计等整个过程中都给予了我悉心的指导,还不惜把自己的研究成果让我参考、借鉴,细心地纠正论文中的错误并给予指导.如果没有他的大力支持,此次论文的完成将变得非常困难.除了敬佩柴老师的专业水平外,他的治学严谨和科学研究的精神也是我永远学习的榜样,然后还要感谢大学四年来所有的老师,为我们打下坚实的专业知识的基础.最后祝各位评审老师身体健康,工作顺利!参考文献[1]数学分析上第三版.华东师范大学数学系编.北京.高等教育出版社,2001,148-154.[2]李惜雯.数学分析例题解析及难点注释(上册).西安.西安交通大学出版社,2004.1,265-269.[3]林源渠方企勤.数学分析解题指南.北京.北京大学大学出版社,2003.11.84-87.[4]大学数学名师导学丛书.北京.中国水利水电出版社,2004208-212..[5]花树忠.邯郸市职工大学基础教学部.邯郸,056001.[6]李世杰.衢州市教育局.浙江.衢州,324002.[7]宋小军.西华师范大学数学与信息学院.四川文理学院学报.2010年5期.[8]陈迪红.长沙铁道学院学报.第12卷.第3期.1994年9月.[9]曹良干.阜阳师范学院学报.总22期.[10]陈太道.琼州大学.数学系.临沂师范学院学报第24卷,第3期.[11]李宗铎.湖南教育学院学报长沙大学.第18卷第2期.。
凸函数在证明不等式中的运用

凸函数在证明不等式中的运用不等式是数学中一个重要的概念,经常出现在数学问题的解决过程中。
而凸函数作为一种特殊的函数类型,具有很好的性质。
本文将介绍凸函数在证明不等式中的运用。
首先,我们来定义凸函数。
在实数集上的函数f(x)被称为凸函数,如果对于任意的x1、x2∈[a,b],以及0≤λ≤1,有:f(λx1+(1-λ)x2)≤λf(x1)+(1-λ)f(x2)这个定义表明,在凸函数上,任意两点的连线实际上都位于函数曲线的上方或切于函数曲线。
凸函数具有很多良好的性质,其中一个非常有用的性质是“切线大于曲线”,即对于凸函数f(x),对于任意x1,x2∈[a,b],有f(x2)≥f(x1)+f'(x1)(x2-x1)。
1.使用切线法。
利用凸函数的“切线大于曲线”性质,我们可以通过构造或应用合适的凸函数来证明各种不等式。
例如,考虑证明柯西-施瓦茨不等式,即:对于任意的a1,a2,…,an和b1,b2,…,bn,有:(a1^2+a2^2+…+an^2)(b1^2+b2^2+…+bn^2)≥(a1b1+a2b2+…+anbn)^2我们可以取凸函数f(x)=x^2,然后应用“切线大于曲线”性质,即:f(b1^2+b2^2+…+bn^2- λ(a1^2+a2^2+…+an^2)) ≥f(b1^2+b2^2+…+bn^2)− λf(a1^2+a2^2+…+an^2)然后,通过选择合适的λ值,并利用普通不等式,我们可以得到柯西-施瓦茨不等式的证明。
2.使用幂平均不等式。
幂平均不等式是一类难题中常见的不等式之一,它可以通过利用凸函数的特性来证明。
根据幂平均不等式的定义,对于任意的正实数x1,x2,⋯,xn和正实数p,q(p≠q),我们有:((x1^p+x2^p+⋯+xn^p)/(n))^(1/p) ≥((x1^q+x2^q+⋯+xn^q)/(n))^(1/q)这个不等式可以通过定义幂函数f(x) = x^p(其中 p>1)来证明。
凸函数的性质在不等式证明中的应用

因此 只需 证 , z 一 l( + l 在 定 义 域 上 是 凸 函 ( ) g 1 O ) ㈣z ∑ 数. 实上 , 平均值不等式 , 事 由 有
寺E (+l ) g1 O2] l 1 O 十l +l )一÷l(+1 + g ( m g1 0t
( ,) 是 上 凸 函数 , 于 任 意 的 z , … z ∈ ( , ) n6内 对 z , 口6 ,
() + z一 彘> ,( 一 g 是 o ) og ) z0z ( 1 z 12 ,
内的下凸函数.
有厂 f
\
±
l t t n
二
成
( 1 )
一
对任意 的 xE( ,) 若 厂 ( ) 0恒 成 立 , _( ) 口6, z≥ 则 厂 z 在
( ,) n 6 内是 下 凸 函 数 ; 若 ( ) 0恒 成 立 , 厂( ) z≤ 则 z 在 ( ,) n 6 内是 上 凸 函数 . 性 质 及 图像 特 征
( ) 如 果 f( ) ( , )内 是 上 凸 函 数 , 有 1 z - 在 n 6 则
( z)
例 2 ( 6年 孝 感 市 二 统 理 科 第 2 0 1题 ) 已知 , z ()
所 以
± L
二
>
i
-
:-O , (号, , (号 号 X CX  ̄ 一 号)E 一 , ) SX O
求: 证
分析 要证: :
≥( ) , 半
≥( , )考 利 可 虑
≥, 丁 x 即证 厂a ) (+ ) ( 3 0 + 一
凸函数及其在不等式证明中的应用

凸函数及其在不等式证明中的应用
凸函数是一种特殊的函数,它是数学及其应用中最重要的函数之一,它具有许多特性,可以用于在约束条件下寻找最优解和不等式证明中。
凸函数具有一个很重要的性质,它满足凸性,即任何两个点之间的夹
线都必须全部位于函数的上方。
这一性质的重要性在于可以将复杂的
问题表示为凸多变量函数的形式,从而使其更容易求解。
在数学分析中,凸性可以用来证明不同的不等式。
def-convex函数的
不等式可以满足:f(x)> a*x + b,其中a,b是算数常数,x是n维向量。
同样,f(x)< a*x + b也可以适用于凸函数,而这正是满足凸函数性质的常见不等式。
此外,凸函数可以用来求解约束优化问题。
如果函数是凸函数,则可
以确保取得最优值,而这样的解决方案也可以用来验证不等式或求解
数学建模问题。
总而言之,凸函数在数学及其应用中十分重要,它的凸性和求解最优
值的能力可用来证明不等式和解决约束优化问题,可以说是一种非常
强大的函数类型。
浅谈凸函数在证明初等不等式中的简单应用

浅谈凸函数在证明初等不等式中的简单应用【摘要】初等不等式证明是高中数学学习阶段的难点之一。
本文介绍了凸函数在证明初等不等式中的简单应用.【关键词】凸函数;不等式初等不等式证明是高中数学学习阶段的难点之一,很多同学为之头痛。
凸函数是一类重要的函数.它的用处很广。
有些不等式利用凸函数的性质、定理来证明非常简洁、巧妙。
下面进行了简单介绍。
*说明:①下文所说凸函数均为下凸函数。
不等号相反,则称是上的上凸函数,不再重述②定理:若数列{an}是递增差数列,则等号成立时{an}是等差数列.1 凸函数的定义及等价定义定义:设函数f(x)在区间[a,b]上有定义,若对[a,b]上任意两点x1,x2,和正数λ∈(0,1),总有:f[λx1+(1+λ)x2]λf(x1)+(1+λ)f(x2)成立,则称f(x)为区间[a,b]上的凸(下凸)函数,若上式仅不等号成立,则称f(x)为区间[a,b]上的严格凸函数.等价定义:设函数f(x)定义在区间I上,如果对x1,x2,有其中λ1>0,λ2>0,且λ1+λ2=1则称f(x)是I上的凸(下凸)函数.2 凸函数的判定定理设f(x)为区间I上的二阶可导函数,则在I上f(x)为凸函数的充要条件是:f(f″(x)0),x∈I3 凸函数的重要性质性质1.(Jensen不等式)若f(x)为区间[a,b]上的凸函数,则对任意xi∈[a,b],λi>0i=1,2,…,n,ni=1λi=1有推论:若f为区间I上的凸函数,则x1,x2…xn∈I必有性质2. (几何特性)f(x)是x∈(x1,x2)上的凸函数,则有:4 凸函数的简单应用有一类不等式利用凸函数的性质、定理来证明非常简洁、巧妙。
证明不等好似就是凸函数的一个应用领域,但关键是构造能够解决问题的凸函数.下面举例说明.例1..若a>0,b>0,p>0、q>1,且1p,1q求证:a1p·b1qap+bq.分析:从所述求证的不等式的形式来看,不容易直接找到合适的凸函数.因此,我们对此不等式进行适当的变形,不妨在不等式的两边同取自然对数,,由此我们就很容易找到合适的凸函数了.证明:考察函数f(x)=-1nx(x>0),因为f″(x)=1x2>0,故f(x)为x>0上的严格凸函数。
凸函数的性质及其在不等式证明中的应用

凸函数的性质及其在不等式证明中的应用凸函数是数学中一个重要的概念,广泛应用于优化理论、经济学、物理学等领域。
在不等式证明中,凸函数可以帮助我们简化证明过程,并且提供了一些常用的不等式。
1. 定义:对于定义在实数域上的函数f(x),如果对于任意的x1、x2,以及0≤t≤1,都有f(tx1+(1-t)x2)≤tf(x1)+(1-t)f(x2),则称函数f(x)是凸函数。
如果不等式方向反过来,即f(tx1+(1-t)x2)≥tf(x1)+(1-t)f(x2),则称函数f(x)是凹函数。
2.一阶导数判别法:如果函数f(x)在区间(a,b)上二次可导,且f''(x)≥0,则f(x)是凸函数;如果f''(x)≤0,则f(x)是凹函数。
3. Jensen不等式:如果函数f(x)是凸函数,则对于任意的实数x1,x2,…,xn,以及任意的正实数λ1,λ2,…,λn,满足λ1+λ2+…+λn=1,有f(λ1x1+λ2x2+…+λnxn)≤λ1f(x1)+λ2f(x2)+…+λnf(xn)。
在不等式证明中,凸函数可以用来简化证明过程,常用的应用有:1. 平均值不等式:对于任意的正实数x1,x2,…,xn,有(x₁+x₂+⋯+xₙ)/n ≥ √(x₁x₂⋯xₙ)。
这个不等式可以通过使用以函数f(x)=ln(x)为代表的凸函数来证明。
由于ln(x)在定义域(0,+∞)上是凸函数,我们可以使用Jensen不等式来证明平均值不等式。
2. Cauchy-Schwarz不等式:对于任意的实数a1,a2,…,an以及b1,b2,…,bn,有(a₁²+a₂²+⋯+aₙ²)(b₁²+b₂²+⋯+bₙ²) ≥(a₁b₁+a₂b₂+⋯+aₙbₙ)²。
这个不等式也可以通过使用凸函数来证明,常用的方法是构造凸函数f(x)=x²,然后应用Jensen不等式。
凸函数的性质及其在不等式证明中的应用

凸函数的性质及其在不等式证明中的应用凸函数是一类在数学中非常重要的函数,它具有很多重要的性质,并且在不等式证明中有着广泛的应用。
在本文中,我将介绍凸函数的性质,并给出一些在不等式证明中的具体应用。
一、凸函数的定义:对于定义在区间上的函数,如果对于区间上的任意两个点和以及任意实数,都有那么我们称函数是凸函数。
如果上式中的等号只在时成立,那么我们称函数是严格凸函数。
二、凸函数的性质:1.凸函数的一阶导数是非递减的。
2.凸函数的二阶导数是非负的。
3.函数的局部极小值点是凸函数。
4.凸函数的和、乘积以及复合仍然是凸函数。
三、凸函数在不等式证明中的应用:凸函数具有很多重要的性质,这些性质使得凸函数在不等式证明中有着广泛的应用。
下面是一些具体的应用示例:1.利用凸函数判断不等式的方向:考虑不等式f(x)≥g(x)如果函数和是凸函数,且在区间上有,那么可以得到f(x) ≥ g(x) for a ≤ x ≤ b2.利用凸函数证明不等式:有时候,我们需要证明一个不等式,其中和可能是一些函数或者表达式。
如果我们可以找到一个凸函数,使得在区间上有,以及在边界处有,那么我们就可以得到f(x) ≥ g(x) for a ≤ x ≤ b从而证明原始的不等式。
3.利用凸函数确定不等式的最优解:在一些优化问题中,我们需要求解一个约束条件下的最优解。
如果我们可以找到一个凸函数,使得在区间上有,且在边界处有,那么我们就可以确定约束条件的最优解。
4.利用凸函数证明柯西不等式:对于实数集和,柯西不等式指的是(a1b1 + a2b2 + ... + anbn)^2 ≤ (a1^2 + a2^2 + ... +an^2)(b1^2 + b2^2 + ... + bn^2)其中和是任意实数。
我们可以通过构造一些凸函数的性质,如二次函数,来证明柯西不等式。
在不等式证明中,凸函数是一个非常重要的工具。
它的性质使得我们可以利用它来判断不等式的方向,证明不等式,确定不等式的最优解,甚至证明柯西不等式等等。
凸函数的性质及其在证明不等式中的应用

凸函数的性质及其在证明不等式中的应用本页仅作为文档页封面,使用时可以删除This document is for reference only-rar21year.March凸函数的性质及其在证明不等式中的应用数学计算机科学学院摘要:凸函数是一类重要的函数.凸函数在不等式的研究中尤为重要,而不等式最终归结为研究函数的特性,这就需要来研究凸函数了.本篇文章论述了凸函数、对数凸函数的定义、引理、定理和性质及其常用的一些判别方法(根据凸函数,对数凸函数的已知的定理、定义、性质,Jensen不等式等一些方法来判断函数是否是凸函数);本文还试就凸函数的等价定义、性质和在证明不等式中的应用等问题作一初步的探讨,以便进一步了解凸函数的性质及其在证明不等式时的作用;并浅谈了一下凸函数在不等式证明中的一些应用(如上述利用凸函数以及对数凸函数的定理,定义,性质,Jensen不等式来证明一些不等式),推广并证明了一些不等式(三角不等式,Jensen不等式等),得到了新的结果.关键词:凸函数;对数凸函数;Jensen不等式;Hadamard不等式;应用Nature of Convex Function and its Application in ProvingInequalitiesChen Huifei, College of Mathematics and Computer ScienceAbstract : Convex function is a kind of important function. Convex function is particularly important in the study of the inequality, and the study of the inequality is reduced to study the characteristics of the convex function,which makes it necessary to study convex functions.We discuss definition, lemma, theorem and the nature of some commonly used discriminant methods of the convex function and the logarithmic convex function in this paper(According to known theorems, definitions, nature, Jensen inequality and other methods of convex function and the logarithmic convex function to recognize whether the function is a convex function); In this paper we also try to discuss the equivalent definition and nature of the convex function and the issue of its application in demonstration inequalities of convex function in order to have a better understanding of the nature and role of the convex function in proving inequalities; we also try to discuss some applications of convex function in proving inequalities(Convex function and the use of these convex function theorem, definition, nature, Jensen inequality to prove Inequality).We also have promoted and proved some inequality (Triangle inequality, Jensen inequality) and reached new results.Key words : Convex function;Logarithmic convex function ; Jensen inequality; Hadamard Inequality;Application1 引言在很多数学问题的分析与证明中,我们都需要用到凸函数,例如在数学分析、函数论、泛函分析、最优化理论等当中.凸函数是一类重要的函数.凸函数在不等式的研究中尤为重要,而不等式最终归结为研究函数的特性,这就需要来研究凸函数了.常用的凸函数有两种,一种叫上凸函数,即曲线位于每一点切线的下方或曲线上任意两点间的弧段总在这两点连线上方的函数;另一种叫下凸函数,即曲线位于每一点切线的上方或曲线上任意两点间的弧段总在这两点连线下方的函数.本文试就凸函数的等价定义、性质和在证明不等式中的应用等问题作一初步的探讨,以便进一步了解凸函数的性质及其作用.2 概念2.1 凸函数的定义上面对凸函数作了直观的描述,我们用分析式子给出其精确定义.定义[1]2.1设函数()f x 在区间[,]a b 上有定义,若对[,]a b 上任意两点12,x x 和正数λ∈(0,1),总有1212[(1)]()(1)()f x x f x f x λλλλ+-≤+- (A)则f 为区间[,]a b 上的凸函数.(同时也称为上凸函数,若是不等号反向则称为下凸函.)定义[1]2.2 若函数()f x 在D 上是正的,且ln ()f x 在D 上是下凸函数,则称()f x 是D 上的对数下凸函数这时, 对于任意,x y D ∈ 和(0,1)λ∈,有ln [(1)]ln ()(1)ln ()f x y f x f y λλλλ+-≤+-. 即(1)[(1)]()()f x y f x f y λλλλ-+-≤ (B)如果(2) 中的不等号反向,则称()f x 是D 上的对数上凸函数.2.2 对数凸函数的性质我们已经有了凸函数以及对数凸函数的定义,现在我们来看一下对数的一些引理,定理及其性质等.定理 2.1[2] (对数下(上) 凸函数的判定定理) 设()f x 是D 上的正值函数,且在D 上有二阶导数,则()f x 在D 上为对数下(上) 凸函数的充要条件为对于任意x ∈D ,有2()()(())0(0)f x f x f x '''-≥≤先证下引理引理 2.1[2] (1) 若()g x 是[,]a b 上的下(上) 凸函数,则()()g x f x e = 为[,]a b e e 上的对数下(上) 凸函数.(2) 若()f x 是[,]c d 上的对数下(上) 凸函数,则()ln ()g x f x =为[ln ,ln ]c d 上的下(上) 凸数.证明(1) 任取12,[,]c d x x e e ∈,由()g x 在[,]c d 上是下凸函数,对任意01λ<<有()()121212[(1)]()(1)()121()()112[(1)][][]()()g x x g x g x g x g x f x x e e e e f x f x λλλλλλλλλλ+-+---+-=≤==(2)任取12,[ln ,ln ]x x c d ∈ ,由()f x 是[,]c d 上的对数下凸函数,对任意01λ<<有11212121212[(1)]ln [(1)]ln[()][()]ln ()(1)ln ()()(1)()g x x f x x f x f x f x f x g x g x λλλλλλλλλλ-+-=+-≤=+-=+-所以()g x 为区间[ln ,ln ]c d 上的下凸函数. (用类似方法可证上凸的情形)下证定理2.1[2] “⇐” 设[,]D c d =,()ln ()g x f x =,则 ()()[ln ()]()f xg x f x f x '''==,22()()[()]()()f x f x f x g x f x '''-''= 所以()g x 是为区间[ln ,ln ]c d 上的下凸函数,根据引理1 得()ln ()()g x f x e e f x ==为[ c ,d] 上的对数下凸函数“⇒” 若()f x 为[,]c d 上的对数下凸函数,由引理1 得()ln ()g x f x =为区间[ln ,ln ]c d 上的下凸函数,从而()0g x ''≥ ,对()ln ()g x f x =求二阶导数即得2()()(())0f x f x f x '''-≥. (用类似方法可证上凸的情形) .推论2.1[2] 设12(),()f x f x 是D 上的对数下(上) 凸函数,则1212()(),()()f x f x f x f x +也是D 上的对数下(上) 凸函数证明:设1212()()(),,,(0,1)g x f x f x x x D λ=+∀∈∈121122121111112221221121122212((1))((1))((1))()()()()[()()][()()]()()g x x f x x f x x f x f x f x fx f x f x f x f x g x g x λλλλλλλλλλλλλλ----+-=+-++-≤+≤+⨯+= 其中(A) 由..H older 不等式得到根据定义 2.2 得出1121()()f x f x +是D 上的对数下凸函数.122112[()()]()()()()f x f x f x f x f x f x '''=+12211212[()()]()()2()()()()f x f x f x f x f x f x f x f x ''''''''=++2121212222221111222[()()][()()]{[()()]}(){()()[()]}(){()()[()]}0f x f x f x f x f x f x f x f x f x f x f x f x f x f x '''-=''''''-+-≥根据定理2.1 得12(),()f x f x 是D 上的对数下凸函数. (用类似方法可证上凸的情形)用数学归纳法可将推论1 推广到有限情形.推论 2.2[2] 设()f x 是定义在D 上的正值函数,1) 若()f x 是对数下凸函数,则1()f x 在区间D 上是对数上凸函数. 2) 若()f x 是对数上凸函数,则1()f x 在区间D 上是对数下凸函数. 证明 1) 设1()()x f x φ=22322224241()()()2(())()(),()[]()()()()()2(())()()()(())()()[()][][][]()()()f x f x f x f x x x f x f x f x f x f x f x f x f x f x f x x x x f x f x f x φφφφφ''''-''''==-=-'''''''--'''-=--=-显然是小于0的,所以1()()x f x φ=是对数上凸函数,同理可证2) . 定理 2.2[2] (Jensen 型不等式) 设()f x 是D 上的正值对数下凸函数, 12,01, (1)i i n x D λλλλ∈<<+++=12112212(...)()()...()n n n n f x x x f x f x f x λλλλλλ+++≤ (*)若()f x 是D 上的正值对数上凸函数,则(*) 中不等号反向.证明 (用数学归纳法) 当2n =时,由定义2.2 知不等式(*) 成立. 假设n k =时不等式(*) 成立,即121122121(...)()()...()(1,0)kkk k k i i i f x x x f x f x f x λλλλλλλλ=+++≤=>∑ ,(1,2,...,1),i x D i k ∈=+设1(1,0)ki i i λλ==>∑111211121111221111111121111211[...()()]()()...()()()()...()()()k k k k k k k k k k k k k k k k k k k k k k k k k k k k k k x x f x x x x x f x f x f x f f x f x f x f x f x λλλλλλλλλλλλλλλλλλλλλλλλλλλ-+-+++--++++++-++-+++++++++≤+≤++ 所以当1n k =+时,不等式(*) 成立,从而对于一切自然数(2)n n ≥ 不等式(*) 成立. 用同样方法可证明上凸情形.当然这里的定理对凸函数也是成立的.在下面的运算性质中有介绍.也就是下面的Jensen 不等式 1,Jensen 不等式 2.引理 2.2[2] (凸函数的Hadamard 不等式) 设()x φ是区间D 上的下凸函数则对于任意,.a b D a b ∈≤有11()[()()]22b a a b x dx a b b aφφφφ+⎛⎫≤≤+ ⎪-⎝⎭⎰ (#) 若()x φ是区间D 上的上凸函数,则对于任意,.a b D a b ∈≤,(#)中不等号反向.定理 2.3[2] ( Hadamard 型不等式) 设():[,](0,)f x a b →+∞对数下凸函数,则11()()[()()]2ln ()ln ()b a a b f f x dx f b f a b a f a f b +≤≤---⎰ (@) 若():[,](0,)f x a b →+∞对数下凸函数,则(5) 中不等号反向. 证明 由引理2.1 和引理2.2有1ln ()ln ()11ln ()()lim lim lim n f a bbf x naan i f a nn n b a f x dx edx e n +∆→∞=+∆→∞→∞-==≥=∑⎰⎰nn 由平均值i=1(b-a )e(b-a )11(ln ())()2lim ()ln ()()()()2ni b aif a bnn b aan a blmf b a ef x dxa bb a eb a f =-+∆-→∞+∑==-+≥-=-⎰1b-a (b-a)e(其中b a ∆=-)又令()ln ()x f x φ=,根据定义2.1,对于a x b <<,有()()()()()a b x b x a x b aφφφ-+-≤-()()()()()()ln ()()()()()()()()()()()exp()|()()[]()()ln ()ln (b a x b a a b x b x a bbbbf x x b aaaaa b a a b b a a b bbb ab aa ab a f x dx edx edx edxb a b a eedx ex b a b a b a b a e e b a f b f a φφφφφφφφφφφφφφφφφ-⎡⎤⎢⎥-⎣⎦-+------==≤--⎡⎤==⎢⎥--⎣⎦--=-=--⎰⎰⎰⎰⎰[()()])f b f a - 定理得证.2.3[3] 凸函数的性质 在讨论了一些对数凸函数的定理,引理,我们来看一看凸函数的运算性质以及它们实用的定理:(1) 若()f x 与()g x 均为区间[,]a b 上的凸函数,则()f x +()g x 也是区间[,]a b 上的凸函数.(2)若()f x 与()g x 为区间[,]a b 上的凸函数,则ⅰ)0λ≥,则()f x λ是[,]a b 上的凸函数;ⅱ)0λ<,则()f x λ是[,]a b 上的凹函数.(3) 设()f x 与()g x 都是[,]a b 上的非负单调递增的凸函数,则()()()h x f x g x =也是[,]a b 上的凸函数.证明:对任意12,x x ∈[,]a b 且12x x <和任意λ∈(0,1),因()f x 与()g x 在[,]a b 上单调递增,故 :1212[()()][()()]0f x f x g x g x --≥即: 12211122()()()()()()()()f x g x f x g x f x g x f x g x +≤+ (1) 又因为()f x 与()g x 在[,]a b 上的凸函数,故1212[(1)]()(1)()f x x f x f x λλλλ+-≤+-,2121g(x +(1-)x )g(x )+(1-)g(x )λλλλ≤而()0,()0f x g x ≥≥,设将上面两个不等式相乘,可得2122222211211[(1)][(1)]()()(1)[()()()()](1)()()f x xg x g x f x f x g x f x g x f x g x λλλλλλλλ+-+-≤+-++-又由⑴知21212222211211[(1)][(1)]()()(1)[()()()()(1)()()]f x x g x x g x f x f x g x f x g x f x g x λλλλλλλ+-+-≤+-++-=1122(1)()()()()f x g x f x g x λλ-+由凸函数的定义知:()()()h x f x g x =是[,]a b 上的凸函数. 注:1°()f x 与()g x 非负不能少,2°(),()f x g x 单调递增不能少.(4)[4][5] 设()u ϕ是单调递增的凸函数,()u f x =是凸函数,则复合函数[()]f x ϕ也是凸函数.对于其他情况也有类似的情况的命题,如下列:我们也可以看一下单值有反函数的函数的反函数与自身的凸凹性的关系. 如下表:(5) 若()f x 为区间I 内的凸函数,且()f x 不是常数,则()f x 在I 内部不能达到最大值.2.4[3] 凸函数的等价定义和判定设函数f 在区间(,)a b 上有定义,则下列命题彼此互相等价:(1)对任意12,x x ∈(,)a b 及任意恒有1212[(1)]()(1)()f x x f x f x λλλλ+-≤+-(2)对任意i x ∈(,)a b 及任意i p >0. 1,2,...,i n =. 11ni i p -=∑ 恒有11()n ni i i i i i f p x p f x ==⎛⎫≤ ⎪⎝⎭∑∑ (3)对任意1,2,(,)x x x a b ∈, 12x x x <<,恒有12121212()()()()()()f x f x f x f x f x f x x x x x x x---≤≤---(4)在(,)a b 上曲线在其每一点处具有不垂直于x 轴的左、右切线,并且曲线在左、右切线之上.(5)若在(,)a b 内存在单调递增的函数()x ϕ.以及0x ∈(,)a b ,使得对任意(,)x a b ∈,恒有00()()()xx f x f x t dt ϕ-=⎰,(6)对任意12,x x ∈(,)a b ,12x x <,恒有21121221()()1()22x x x x f x f x f f t dt x x ++⎛⎫≤≤ ⎪-⎝⎭⎰(7)对任意12,(,)x x a b ∈,恒有1212()()22x x f x f x f ++⎛⎫≤ ⎪⎝⎭对于凸函数定义等价性的证明,可参看[4]及[5].对于等价定义(5)事实上,我们也有类似的这样一个定理:定理 2.4 设函数f 在[,]a b 上连续,在(,)a b 上可导,则f 在[,]a b 上为上(下)凸函数(严格上(下)凸函数)的一个必要充分条件f '是在(,)a b 上递增(减)(严格递增(减)).证明 先证条件是必要的.设()12,(,)x x a b ⊂.只要x x '与满足12x x x x '<<<,由于等价定义(3)可知12121212()()()()()()f x f x f x f x f x f x x x x x x x '---≤≤'---在上式中令12,x x x x +-'→→,得211221()()()()f x f x f x f x x x -''≤≤-.在是严格上凸函数的情形,我们取一点*x 满足*12x x x <<,从而得出**1212**12()()()()()()f x f x f x f x f x f x x x x x --''≤<≤--. 这样就得出了严格的不等式12()()f x f x ''<,必要性得证.再证充分性.设f '是在(,)a b 上递增.对任何()12,x x x ∈,由Lagrange 中值定理,可只存在()12,x x ξ∈与()12,x x η∈,使得11()()()f x f x f x x ξ-'=-,22()()()f x f x f x xη-'=-因为x ξη<<,所以()()f f ξη''≤.从而有1212()()()()f x f x f x f x x x x x--≤--所以,可知函数f 在[,]a b 上为上凸函数.容易看出,当f '严格递增时,()()f f ξη''<.上述不等式中成立着严格的不等号,从而函数f 在[,]a b 上是严格的上凸函数.同理可以证明下凸时的情景.当函数f 在[,]a b 内有二阶导数时,我们有下列应用起来就会更方便的定理 定理 2.5 设函数f 在[,]a b 上连续,f 在(,)a b 内有二阶导数,则f 在[,]a b 上为上凸函数(下凸函数)的充分条件0(0)f f ''''≥≤在(,)a b 内成立;而f 在[,]a b 上为严格上(下)凸函数的充分必要条件是0(0)f f ''''≥≤在(,)a b 内成立并且在(,)a b 的任何开的子区间内f ''不恒等于0.证明 第一个结论,由于0f ''≥得出f '在(,)a b 上递增再由定理4可得出.同理可证明下凸时的情景; 第二个结论,先证充分性 由于0f ''≥在(,)a b 内成立并且在(,)a b 的任何开的子区间内f ''不恒等于0.对任意12,(,)x x a b ∈,12x x <,又由于2121()()()x x f x f x f x dx ''''=+⎰,所以21()()f x f x ''>.所以函数f 在[,]a b 上为严格的凸函数.充分性得证. 再证必要性(反证法) 因为函数f 在[,]a b 上为严格凸函数,对任意12,(,)x x a b ∈,12x x <,则21()()f x f x ''>,而由于2121()()()x x f x f x f x dx ''''=+⎰,若是有一个(,)a b 的子区间恒等于0.不妨设为(,)(,)a b ξη⊂,对任意(,)x ξη∈,()0f x ''=.则由于21()()()x x f f f x dx ηξ''''=+⎰,()()f f ξη''=,这与已知条件相矛盾.所以,必要性得证.同理可证明下凸时的情景. 所以,定理得证.关于凸函数的判定有很多,应用范围最广的是Jensen 不等式.Jensen 不等式 1 设()f x 在区间I 上有定义,()f x 为凸函数,当且仅当12,,...,n x x x I∀∈1212...()()...()n n x x x f x f x f x f n n ++++++⎛⎫≤⎪⎝⎭(J1) 此外,当且仅当12...n x x x === 时,上式等号成立(证明略请参考附[1]). Jensen 不等式 2 12,,...,[,]n x x x a b ∀∈,12,,...,0n λλλ>,且11ni i λ==∑,1.则()f x 为凸函数的充要条件为:11()()n ni i i i i i f x f x λλ==≤∑∑ (J2)此外,上式当且仅当12...n x x x === 时,等号成立.(证明略请参考附[1]). 这里对任意12,,...,0n βββ>,若是令1ii nii βλβ==∑,那么就有1111()nni i i i i i n n i i i i x f x f ββββ====⎛⎫ ⎪ ⎪≤⎪ ⎪⎝⎭∑∑∑∑ (J3) 每个凸函数都有一个Jensen 不等式,Jensen 不等式的应用范围甚广,既可用于求解不等式问题,又可用于证明不等式定理,应用Jensen 不等式解题的关键有两条:一是必须先判明函数的上(下)凸性,二是直接应用Jensen 不等式有困难时,可以根据命题的特点,选择恰当的上凸函数和下凸函数,然后再进行解答.3 凸函数以及对数凸函数的应用在许多证明题中,我们常常遇到一些不等式的证明,其中有一类不等式利用凸函数的性质来证明可以非常简洁、巧妙.证明不等式是凸函数的一个重要应用领域,但关键是构造能够解决问题的凸函数.例 1[1] 利用凸函数证明调和平均值H ≤几何平均值G ≤对数平均值L ≤指数平均值E ≤算术平均值A.证明:事实上,我们可以用凸函数理论证明,对任意0(1,2,...,)ix i n 有1212...111...nnx x x n nx x x +++≤≤+++ (2)只要将不等式各部分同时取对数,这时左边的不等式可变为121111...1111ln (ln ln ...ln )n nx x x n n x x x +++-≤----.从而由函数()ln f x x =-在(0,)+∞上的(严格)凸性可得;右边的不等式可直接由()ln g x x =上的(0,)+∞(严格)下凸性可得.(具体证明可参看[2])为了证明例1 中的连不等式,我们先来看下面两个小题:(1) 设0(1,2,...,)i a i n >=且不全相等,0(1,2,...,)i p i n >=有不等式链11111ln ln exp exp n n nii i i i i i i i i nn n ii i i i n i i p a p a p a a p p p a ======⎛⎫⎛⎫ ⎪ ⎪ ⎪≤≤ ⎪ ⎪ ⎪ ⎪⎪⎝⎭⎝⎭∑∑∑∑∑∑ (3) 证:凸函数()ln f x x =-的Jensen 不等式:取0i q >,11ni i q ==∑,0(1,2,...,).i a i n >=得11ln ln n n i i i i i i q a q a ==-≤-∑∑ [4] 111ln ln nni i i i i i q q a a ==-≤-∑∑ (5)在[4]中令1iini ii ip a q p a ==∑得 1111exp ln nn niiii ni i i i iii ip p p a p a a a ====⎛⎫≤ ⎪⎝⎭∑∑∑∑ (6)又由(4),(5)可得 1111in nq i i i n i i i i ia q a q a ===≤≤∑∏∑ (7)在此令1ini i i p q p ==∑,可得111111ln exp nn ni i i i ii i i n n n ii i i i i ip p a p a p p p a ======⎛⎫ ⎪≤≤ ⎪ ⎪ ⎪⎝⎭∑∑∑∑∑∑ (8)联立(6),(8)既得证 (3).(2) 设()()f x p x 与在[,]a b 上正的连续函数且()f x ≠常数,在⑻中作代换i b a p p a i n -⎛⎫=+ ⎪⎝⎭,i b a a f a i n -⎛⎫=+ ⎪⎝⎭并在“∑”号后均乘b a n -,由0b a ->,不改变原不等号方向.令n →∞ 便得(3)的积分形式:ln ln exp exp b bb ba aa ab b bba aa ap fdx pdxp fdx pfdx f p p pdx pdxdx dx f f ⎛⎫⎛⎫ ⎪⎪ ⎪≤≤≤ ⎪ ⎪ ⎪ ⎪⎝⎭⎝⎭⎰⎰⎰⎰⎰⎰⎰⎰(3)'在(3)'中令()1,()p x f x x ==()11ln ln ln ln 2b ab a b a b ab a e ----+⎛⎫≤≤≤⎪-⎝⎭再联立(2),得出H G L E A ≤≤≤≤.例 2 (1)在锐角ABC ∆中,证明1cos cos cos 2A B C ++≤, (2)12,,...,n a a a 设为正数,证明恒成立12...n a a a n +++≥证明 (1)令()cos()f x x =-,(0,)x π∈.由于()cos()0f x x ''=>,(0,)2x π∈.所以()f x 在(0,)2x π∈上凸函数,所以由于(J1)()()()()33f A f B f C A B C f ++++≥,即cos()cos()cos()s()33A B C A B C co ---++≥-1()2=-即1cos cos cos 2A B C ++≤;(2) 令()ln ,(0,)g x x x =-∈+∞,所以21()0,(0,)g x x x''=>∈+∞,故()g x 是在(0,)+∞上的上凸函数.也是根据(J1)121212121212()()...()...()ln ln ...ln ...ln()ln ln ...ln ...ln()n nn nn n g a g a g a a a a g n n a a a a a a n na a a a a a n n++++++≥++++++-≥-++++++≤即即从而,有12...n a a a n+++≥下面我们再看一个用对数凸函数证明的不等式题. 例 3[2]10,0,12ni i i πλλ=<<>=∑i 设x ,则12112212sin(...)sin sin ...sin n n n n x x x x x x λλλλλλ+++≥ (&)12112212cos(...)cos cos ...cos n n n n x x x x x x λλλλλλ+++≥ (%)证明 设()sin()f x x =,由于2()()[()]10f x f x f x '''-=-<,故sin()x 是(0,)2π上的对数凸函数,同理cos()x 也是(0,)2π上对数凸函数.根据定理2即可得(&),(%).例 4 设()f x 在[,]a b 上可积,且()m f x M ≤≤,()t ϕ是在[,]m M 上的连续下凸函数,则11()(())b b a af x dx f x dx b a b a ϕϕ⎛⎫≥ ⎪--⎝⎭⎰⎰. 证明 令,()k n k f f a b a n ⎛⎫=+- ⎪⎝⎭,,1()k n x b a n ∆=-.由于()t ϕ是凸函数,故有1,2,,1,2,,...()()...()n n n n n n n n f f f f f f n n ϕϕϕϕ++++++⎛⎫≥⎪⎝⎭. 由定积分的定义,上式就相当于,,,,11()n ni n i n i n i ni i f f b a b a ϕϕ==⎛⎫∆∆ ⎪ ⎪≥-- ⎪⎪⎝⎭∑∑,,1()k n x b a n ∆=-在上式中令n →∞时, 则有11()(())b b a a f x dx f x dx b a b a ϕϕ⎛⎫≥ ⎪--⎝⎭⎰⎰. 命题得证.例 5[7]设,i i a b R +∈,111,2,...,,,n n i i i i i n a b ====∑∑则21112nni i i i i ia a ab ==≥+∑∑.证明 记1ni i s a ==∑,11ni i a s ==∑,将21112nni i i i i i a a a b ==≥+∑∑变为11121n ii i ia b s a =≥+∑,那么取11i ib a +作为函数1()1f x x=+,则由于3()2(1)0f x x -''=+>,再令i i i b x a =,ii a sλ=所以根据凸函数性质和(J3)得出11111211ni n i i i ii i a b s x a λ==≥=++∑∑结论本文主要讨论了凸函数以及对数凸函数一类重要的函数的概念,包括它们的一些定义,性质,定理,引理和它们在证明一些不等式的重要应用.本文介绍了Jensen 不等式,Hadamard 不等式,叙述了一些定理,引理,性质并给出了它们的证明,并指出它们在判断凸函数的应用.本文还试就凸函数的等价定义、性质和在证明不等式中的应用等问题作一初步的探讨,以便进一步了解凸函数的性质及其在证明不等式时的作用.最后举出了一些例题来具体的来体现凸函数以及对数凸函数在不等式证明的应用.参考文献:[1]汪文珑.数学分析选讲[M].绍兴文理学院数学系,2001[2]刘琼.对数凸函数的Jensen型和Hadamard型不等式[J].邵阳学报,邵阳,2005,3[3]查良凇.凸函数及其在不等式证明中的应用[J].浙江工贸职业技术学院学报,绍兴,2005,3[4]燕建梁,张喜善.凸函数的性质及其在不等式证明中的应用[J].太原教育学院学报,太原,2002,4[5]T.M菲赫金哥尔茨普.微积分教程[M].1965: 290-300[6]常庚哲,史济怀.数学分析教程(上册)(M).高等教育出版社,2003:167-176[7]李碧荣.凸函数及其性质在不等式证明中的应用[J].广西师范学院学报,南宁,2004,2[8]白景华.图函数的性质、等价定义及应用[J].开封大学学报,开封,2003,2[9]Satish Shirali, Harkrishan L. Vasudeva. Mathematical analysis[M]. Alpha Science International Ltd., c2006.[10]Tom M. Apostol.Mathematical analysis[M].China Machine Press, 2004.致谢这是本人的第一篇论文,所以在多方面没有指导老师张金洪老师的指导是很难进行下去的.张老师从我的选题开始便给予了很大帮助,在以后的开题,开题报告,初稿的资料搜索,初稿出来后的校正,进一步的改进都给予了极大帮助,使我在论文的完成进程中得以较为平坦地进行下去.在论文的写作的进行中,我同组等同学也给了我很多帮助.在此表示感谢.也在此对我们的学校安徽师范大学以及我校资料室提供这样一个学习环境和帮助,表示感谢.也感谢那在身后的帮助.。
凸函数的性质及其在证明不等式中的应用

凸函数的性质及其在证明不等式中的应用凸函数(Convex function)是数学中的一种特殊函数,具有一些特殊的性质和应用。
在证明不等式中,凸函数的性质可以帮助我们简化问题,提供了一种有效的方法。
1. 定义:对于定义在实数集上的函数f(x),如果对于任意的x1,x2∈R以及0≤t≤1,都有f(tx1+(1-t)x2)≤tf(x1)+(1-t)f(x2),那么f(x)是凸函数。
2.几何意义:凸函数的几何意义可以通过以下两点来理解。
首先,凸函数的图像上的任意两点形成的线段在函数图像的上方或者处于函数图像上。
其次,凸函数的下方的切线都位于函数图像下方。
3.一阶导数条件:对于凸函数来说,一阶导数是单调递增的。
也就是说,如果f(x)是凸函数,则f'(x)≥0。
4.二阶导数条件:凸函数的二阶导数是非负的。
也就是说,如果f(x)是凸函数,则f''(x)≥0。
凸函数在证明不等式中的应用:1.约束条件:凸函数在一些约束条件下的最大值或最小值通常是问题的关键。
我们可以通过构造一个约束函数和一个目标函数,来求解最优化问题。
通常情况下,约束函数是一个凸函数,而目标函数是可以转化为凸函数的。
2.差分近似:在证明不等式过程中,我们常常需要利用凸函数近似一些复杂的函数。
这是因为凸函数在大部分区间上是递增的,所以可以将复杂的问题简化为凸函数问题。
3. Jensen不等式:Jensen不等式是证明凸函数不等式的重要工具。
Jensen不等式指出,如果f(x)是凸函数且x1, x2, ..., xn是任意实数,那么有f(λ1x1+λ2x2+...+λnxn) ≤λ1f(x1)+λ2f(x2)+...+λnf(xn),其中λ1, λ2, ..., λn是非负实数且满足λ1+λ2+...+λn=14. Karamata不等式:Karamata不等式是一种更加广义的不等式,可以被用于证明许多重要的几何不等式。
这个不等式是基于对凸函数定义的一个扩展。
应用凹凸函数的性质证明不等式解读

sin Α+co s Α
2
=1+2sin2Α+
4
2sin (Α+
Π4
≥1+2+4
2
=3+2 2.
例2已知A 1,A 2,A 3,…,A n是凸n边形的n个内角.求证:
sin A 1+sin A 2+…+sin A n ≤n sin (n -2Π
n
.
证明 由平面几何知识可知A i ∈
(0,Π,i =1,2,3,…,n ,且A 1+A 2+…+A n
f (x 2≤2f (
x 1+x 2
2
(当且仅当x 1=x 2时取等号,则称f (x在[a ,b ]上是凸函数;若恒
有f (x 1+f (x 2≥2f (
x 1+x 2
2
(当且仅当x 1=x 2时取等号,则称f (x在[a ,b ]上是凹函数.
应用数学归纳法,我们可以证明下面的凹(或凸函数的性质.
定理 若函数f (x在某区间内是凹(或凸函数,则对变数在这区间内的任意值x 1,x 2,x 3,…x n有以下不等式成立:
一般的随机事件,用统计定义求出它的概率,需要做多次实验(而且还不能找出精确值.为此,对实验合理的设计,数据的处
论:
当x1,x2,…,x n∈R+,且x1+x2+…+ x n=1时,则有
(x1+1
x12+(x2+1
x2
2+…+(x n+1
x n
2
≥(n2+12
n
.
例4设a、b、c为△A B C的三边,S是
凸函数及其在证明不等式中的应用

凸函数及其在证明不等式中的应用凸函数是数学分析中的重要概念,它在不等式的证明中发挥着重要作用。
本文将介绍凸函数以及它在证明不等式中的应用。
凸函数是一个定义在实数轴上的函数,它的一个重要特性是对于函数上任意两点,连接这两点的弦不会低于函数上的任意一点。
凸函数的形象化理解是函数图像位于对应的弦的上方。
具体定义上,设函数f(x)在区间[a,b]上有定义,如果对于任意的x1,x2∈[a,b]以及任意介于x1和x2之间的t∈[0,1],有f(tx1 + (1-t)x2) ≤ tf(x1) + (1-t)f(x2)则称函数f(x)在区间[a,b]上是凸函数。
凸函数具有很多重要的性质,这些性质在证明不等式中起到了关键作用。
下面将介绍几个常见的凸函数的性质。
首先,凸函数的导函数递增。
也就是说,如果f(x)是一个凸函数,则f'(x)≥0。
这个性质可以用凸函数的定义来证明。
假设存在x1,x2∈[a,b],且x1<x2,使得f'(x1)>f'(x2)。
根据导函数的定义,可以得到f'(x1) = lim┬(h→0)(f(x1+h)-f(x1))/hf'(x2) = lim┬(h→0)(f(x2+h)-f(x2))/h由于f'(x1)>f'(x2),因此存在一个Δ>0,对于任意的0<h<Δ,均有(f(x2+h)-f(x2))/h≤(f(x1+h)-f(x1))/hf(x2+h)-f(x2)≤f(x1+h)-f(x1)由此可得f(x2)≤f(x1),与凸函数的定义矛盾,因此f'(x)必须递增。
其次,凸函数的二阶导函数非负。
也就是说,对于凸函数f(x),有f"(x)≥0。
这个性质的证明可以通过分别计算f(x)的一阶导函数和二阶导函数来完成。
如果一阶导函数f'(x)≥0,那么f(x)是递增函数,因此它的二阶导函数f"(x)≥0。
凸函数及其在不等式证明中的应用

凸函数及其在不等式证明中的应用摘要:凸函数是一类重要的函数,在数学许多问题中都有广泛的应用。
本文论述了凸函数的定义、性质及其判别方法,讨论了凸函数在不等式证明中的重要应用并对凸函数进行了推广。
关键词:凸函数; 性质; 不等式; Jensen不等式Convex Function and its Application in the proof InequalityAbstract Convex Function is a kind of important Function, it has a far-ranging application in a lot of mathematical problems .The paper related and analyzed the definition,property, and discriminant method of the convex Function .At the same time,the theme talked about the Convex Function’s important in the proof Inequality and popularized about the Convex Function.Key Words Convex Function; property; Inequality; Jensen Inequality目录题目:凸函数及其在不等式证明中的应用 (1)摘要 (1)关键词 (1)引言 (1)1凸函数的定义、性质及判定定理 (1)1.1凸函数的定义 (1)1.2凸函数的几种等价定义 (2)1.3凸函数的性质及定理 (3)2关于凸函数的四个不等式 (4)2.1 Jensen不等式1 (4)2.2 Jensen不等式2 (4)2.3 Holder不等式1 (5)2.4 Holder不等式2 (6)3凸函数在不等式证明中的应用 (7)3.1利用Jensen不等式1和凸函数性质证明不等式 (7)3.2利用Jensen不等式2和凸函数性质证明不等式 (9)3.3凸函数在积分不等式中的应用. (10)4凸函数的推广 (11)4.1凸函数的定义推广 (11)4.2凸函数的性质及定理推广 (12)4.2.1凸函数的性质推广 (12)4.2.2凸函数的定理推广 (13)结束语 (14)参考文献 (15)致谢 (16)凸函数及其在不等式证明中的应用王红娟(天水师院 数学与统计学院 甘肃 天水 741000)摘 要: 凸函数是一类重要的函数,在数学许多问题中都有广泛的应用。
凹凸函数的性质在不等式证明中的应用

凹凸函数的性质在不等式证明中的应用凹凸函数是数学分析中的重要概念,在不等式证明中有着广泛的应用。
凹凸函数在不等式证明中的应用可以帮助我们更精确地估计函数的取值范围,以及确定不等式的成立条件。
下面将分别从凸函数和凹函数两个方面来讨论凹凸函数在不等式证明中的应用。
首先,我们来解释凸函数和凹函数的定义:设函数f(x)在区间I上连续,如果对于区间I上的任意两点x1和x2以及任意t∈[0,1],都有以下不等式成立:f(tx1+(1-t)x2)≤tf(x1)+(1-t)f(x2),这样的函数称为凸函数;f(tx1+(1-t)x2)≥tf(x1)+(1-t)f(x2),这样的函数称为凹函数。
接下来,我们将讨论凸函数在不等式证明中的应用。
1.凸函数在不等式证明中的应用:凸函数在区间上的取值比割线的取值更小。
这个性质被称为下凸性。
具体来说,如果函数f(x)在区间I上是凸函数,对于区间上的任意两点x1和x2,都有以下不等式成立:f(x)≥f(x1)+f′(x1)(x-x1),其中f′(x)表示函数f(x)的导数。
基于凸函数的这个性质我们可以得到以下结论:1.1瑕疵卡西:设函数f(x)在区间I上是凸函数,则对于区间I上的任意两点,有:f(x1+x2)≥f(x1)+f(x2),即凸函数的和大于等于函数的和。
1.2 杨辉三角形不等式:设函数f(x)在区间I上是凸函数,则对于区间I上的任意n个实数x1, x2, ..., xn,有:f(x1+x2+...+xn)≥f(x1)+f(x2)+...+f(xn) ,即凸函数的和大于等于函数的和。
1.3 杨辉不等式:设函数f(x)在区间I上是凸函数,则对于区间I 上的任意n个不等的实数x1, x2, ..., xn,有:f((x1+x2)/2)+f((x2+x3)/2)+...+f((xn-1+xn)/2)≤(f(x1)+f(x2)+...+f(xn))/(n-1) ,即凸函数的均值小于等于函数的均值。
凸函数在不等式中的证明

凸函数在不等式中的证明1.函数的定义及其常见的凹凸函数大家都熟悉函数2()f x x =的图像,它的特点是:曲线2y x =上任意两点间的弧总在这两点连线的下方。
我们可以下这样一个定义:设()f x 在[,]a b 上有定义,若曲线()y f x =上任意两点间的弧总位于连接该两点的直线之下,则称函数()f x 是凸函数.上面的定义只是几何描述性的,为了便于凸函数的应用,用严格的式子分析定义凸函数是十分必要的.在不等式的证明中经常会应用到凸函数的两个定义:定义1 设()f x 在(,)a b 内连续,如果对(,)a b 内任意两点12,x x 恒有 1212()()()22x x f x f x f ++≤ 那么称()f x 在(,)a b 内是凸函数.定义2 设()f x 在(,)a b 内连续,如果对(,)a b 内任意两点12,,(0,1)x x λ∈ ,有 )()1()())1((2121x f x f x x f λλλλ-+≤-+ 则称()f x 在(,)a b 内是凸函数.以上若不等式的方向相反,则称()f x 在(,)a b 内是凹函数.1.1常见的凹凸函数有1.1.1 )0()(<=k x x f k 或)0(>k ,x x x f ln )(=均为(0,)∞内的严格凸函数;1.1.2 ()ln(1),()0)x f x e f x c =+=≠均为(,)-∞+∞内的严格凸函数.1.2 凸函数的常见性质及其判定定理性质1 设()f x 为凸函数,0k >为常数,则()kf x 是凸函数:若()(1,2,...,)i f x i n = 是凸函数,则1()ni i f x =∑ 仍是凸函数:若()u ϕ是增凸函数,()u f x =也是凸函数,则复合函数[()]f x ϕ也是凸函数[1].性质2 如果()f x 是(,)a b 上的凸函数,则在(,)a b 的任一闭子区间上有界.性质3 如果()f x 是(,)a b 上的凸函数,则()f x 在(,)a b 内连续.定理1[1]()f x 是区间I 上的凸函数的充要条件是:对于满足11ni i λ==∑ 的任意12,,...,0n λλλ≥ ,有:11()()n n i i i i i i f x f x λλ==≤∑∑ 12,,...,n x x x I ∀∈ (1)定理2 若()f x 在区间I 上二阶可微,则()f x 在I 上是凸函数的充要条件是:1.3凸函数的不等式 1.3.1 凸函数基本不等式设()f x 是(,)a b 内的严格凸(凹)函数,则对(,)a b 内的任意一组不全相同的值12,,...,n x x x ,必有不等式[2]:1.3.2 Jensen 不等式Jensen 不等式是凸函数的一个重要性质,利用其证明一些重要不等式可以更简捷,它有如下两种形式:(1) 设()f x 是(,)a b 内的凸(凹)函数,则对(,)a b 内的任意一组值12,,...,n x x x 及任意正数12,,...,n p p p 必有不等式: 112211221212...()()...()()()......n n n n n np x p x p x p f x p f x p f x f p p p p p p ++++++≤≥++++++ (2)设(),()f x p x 为[,]a b 上的可积函数,而 (),()0,()0ba m f x M p x p x dx ≤≤≥>⎰则当()()t m t M ϕ≤≤为凸(凹)函数时有()()()[()]()()()()bbaabbaap x f x dxp x f x dxp x dxp x dxϕϕ≤≥⎰⎰⎰⎰2.凸函数在证明不等式中的简单应用在初等数学中,调和平均值不大于几何平均值,几何平均值不大于算术平均值,算术平均值不大于平方平均值,而证明用到数学归纳法.其实,这些不等式可在凸函数框架下统一证明.例1 设0,1,2,...,i a i n >= ,证明:1212...111...nna a a n na a a +++≤≤+++证明 设()ln ,(0,)f x x x =-∀∈∞ ,有01)(2''>=x x f ,从而,函数()ln f x x =-在(0,)∞是严格凸函数, 取121(0,),,1,2,...,,...1i i i n x a q i n q q q n=∈∞==+++=有1212ln ln ln ln(...)...n n a a a a a a n n n n n n-+++≤----或n n n n n n na a a a a a na a a ...ln )ln ...ln (ln ...ln 211121121-=+++-≤+++- 即12...na a a n+++≤取 1211(0,),,1,2,...,,...1i i n i x q i n q q q a n=∈∞==+++= 同样方法,有12...nn a a a ≤+++于是,n N +∀∈ , 有1212......nna a a n na a a +++≤≤+++例2 证明12,,...,,1n x x x R p +∀∈≥ 有 11212......()p p p pn n x x x x x x n n++++++≤上式称为算术平均不大于(1)p p ≥ 次平均,特别的,当2p = ,得到算术平均值不大于平方平均值。
凸函数及其性质在不等式证明中的应用

凸函数及其性质在不等式证明中的应用
凸函数是数学中重要的一个分支,它的性质直接关系到许多应用中的非线性规划问题的求解。
它的作用横跨金融、优化运筹、机器学习等多个领域,能够极大地提高工作效率和解决方案质量,是越来越受到重视的数学工具。
在不等式证明这一领域,凸函数的优势表现得淋漓尽致。
它可以快速、准确地解决诸如约束最优化等复杂的数学模型,使收敛性更高、解决效果更佳,从而大大提高工作效率。
此外,它还可以有效、精确地计算任意函数的边界,并可以根据实际情况求解凸集或凸函数的局部参数最优化问题。
凸函数还有一个重要的特点,即它的近似性可以在一定程度上控制在可接受范围内。
这一特点与一般的函数相比,在处理复杂场景时有着重要的意义,可有效减少误差,从而获得更准确的结果。
凸函数具备多种强大的优势,同时也是一种理想的数学工具,可以最大限度地满足一般不等式证明所需的要求。
因此,强烈推荐从事不等式证明研究的专家学者们使用凸函数,作为解决复杂问题的利器,可以从根本上有效解决约束最优化等复杂的算法问题,实现更高效的计算证明。
运用凸函数性质证明不等式
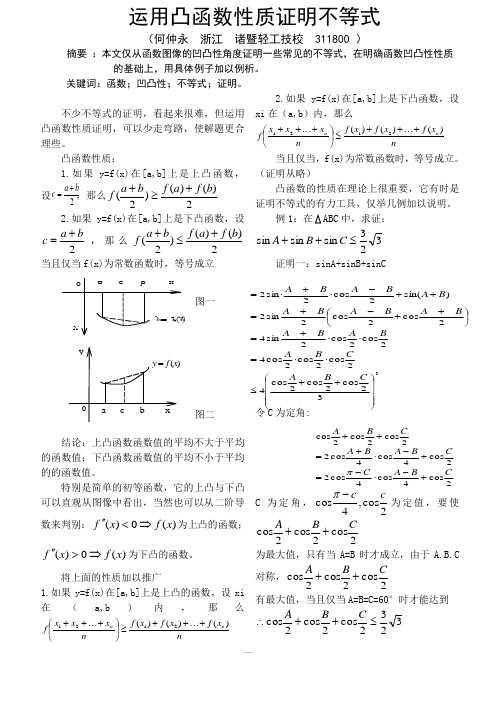
不少不等式的证明,看起来很难,但运用凸函数性质证明,可以少走弯路,使解题更合理些。
凸函数性质:1.如果y=f(x)在[a,b]上是上凸函数,设 ,那么2)()()2(b f a f b a f +≥+ 2.如果y=f(x)在[a,b]上是下凸函数,设2b a c +=,那么2)()()2(b f a f b a f +≤+ 当且仅当f(x)为常数函数时,等号成立结论:上凸函数函数值的平均不大于平均的函数值;下凸函数函数值的平均不小于平均的的函数值。
特别是简单的初等函数,它的上凸与下凸可以直观从图像中看出,当然也可以从二阶导数来判别:)(0)(x f x f ⇒<''为上凸的函数;)(0)(x f x f ⇒>''为下凸的函数。
将上面的性质加以推广1.如果y=f(x)在[a,b]上是上凸的函数,设xi在(a,b )内,那么n x f x f x f n x x x f n n )()()(2121+⋯++≥⎪⎭⎫ ⎝⎛+⋯++ 2.如果y=f(x)在[a,b]上是下凸函数,设xi 在(a,b )内,那么n x f x f x f n x x x f n n )()()(2121+⋯++≤⎪⎭⎫ ⎝⎛+⋯++ 当且仅当,f(x)为常数函数时,等号成立。
(证明从略)凸函数的性质在理论上很重要,它有时是证明不等式的有力工具,仅举几例加以说明。
例1中,求证:323sin sin sin ≤++C B A 证明一:sinA+sinB+sinC令C 为定角:C 为定角,2cos ,4coscc-π为定值,要使2cos 2cos 2cos CB A ++为最大值,只有当A=B 时才成立,由于A.B.C对称,2cos 2cos 2cos CB A ++ 有最大值,当且仅当A=B=C=60°时才能达到3232cos 2cos 2cos≤++∴C B A 2b a c +=运用凸函数性质证明不等式(何仲永 浙江 诸暨轻工技校 311800 )摘要 :本文仅从函数图像的凹凸性角度证明一些常见的不等式,在明确函数凹凸性性质的基础上,用具体例子加以例析。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
凸函数的性质及其在证明不等式中的应用数学计算机科学学院摘要:凸函数是一类重要的函数.凸函数在不等式的研究中尤为重要,而不等式最终归结为研究函数的特性,这就需要来研究凸函数了.本篇文章论述了凸函数、对数凸函数的定义、引理、定理和性质及其常用的一些判别方法(根据凸函数,对数凸函数的已知的定理、定义、性质,Jensen不等式等一些方法来判断函数是否是凸函数);本文还试就凸函数的等价定义、性质和在证明不等式中的应用等问题作一初步的探讨,以便进一步了解凸函数的性质及其在证明不等式时的作用;并浅谈了一下凸函数在不等式证明中的一些应用(如上述利用凸函数以及对数凸函数的定理,定义,性质,Jensen不等式来证明一些不等式),推广并证明了一些不等式(三角不等式,Jensen不等式等),得到了新的结果.关键词:凸函数;对数凸函数;Jensen不等式;Hadamard不等式;应用Nature of Convex Function and its Application in ProvingInequalitiesChen Huifei, College of Mathematics and Computer ScienceAbstract : Convex function is a kind of important function. Convex function is particularly important in the study of the inequality, and the study of the inequality is reduced to study the characteristics of the convex function,whichmakes it necessary to study convex functions.We discuss definition, lemma, theorem and the nature of some commonly used discriminant methods of the convex function and the logarithmic convex function in this paper(According to known theorems, definitions, nature, Jensen inequality and other methods of convex function and the logarithmic convex function to recognize whether the function is a convex function); In this paper we also try to discuss the equivalent definition and nature of the convex function and the issue of its application in demonstration inequalities of convex function in order to have a better understanding of the nature and role of the convex function in proving inequalities; we also try to discuss some applications of convex function in proving inequalities(Convex function and the use of these convex function theorem, definition, nature, Jensen inequality to prove Inequality). We also have promoted and proved some inequality (Triangle inequality, Jensen inequality) and reached new results.Key words : Convex function;Logarithmic convex function ; Jensen inequality; Hadamard Inequality;Application1引言在很多数学问题的分析与证明中,我们都需要用到凸函数,例如在数学分析、函数论、泛函分析、最优化理论等当中.凸函数是一类重要的函数.凸函数在不等式的研究中尤为重要,而不等式最终归结为研究函数的特性,这就需要来研究凸函数了.常用的凸函数有两种,一种叫上凸函数,即曲线位于每一点切线的下方或曲线上任意两点间的弧段总在这两点连线上方的函数;另一种叫下凸函数,即曲线位于每一点切线的上方或曲线上任意两点间的弧段总在这两点连线下方的函数.本文试就凸函数的等价定义、性质和在证明不等式中的应用等问题作一初步的探讨,以便进一步了解凸函数的性质及其作用.2 概念2.1 凸函数的定义上面对凸函数作了直观的描述,我们用分析式子给出其精确定义.定义[1]2.1设函数()f x 在区间[,]a b 上有定义,若对[,]a b 上任意两点12,x x 和正数λ∈(0,1),总有1212[(1)]()(1)()f x x f x f x λλλλ+-≤+- (A)则f 为区间[,]a b 上的凸函数.(同时也称为上凸函数,若是不等号反向则称为下凸函.)定义[1]2.2 若函数()f x 在D 上是正的,且ln ()f x 在D 上是下凸函数,则称()f x 是D 上的对数下凸函数这时, 对于任意,x y D ∈ 和(0,1)λ∈,有ln [(1)]ln ()(1)ln ()f x y f x f y λλλλ+-≤+-. 即(1)[(1)]()()f x y f x f y λλλλ-+-≤ (B)如果(2) 中的不等号反向,则称()f x 是D 上的对数上凸函数.2.2 对数凸函数的性质 我们已经有了凸函数以及对数凸函数的定义,现在我们来看一下对数的一些引理,定理及其性质等.定理 2.1[2] (对数下(上) 凸函数的判定定理) 设()f x 是D 上的正值函数,且在D 上有二阶导数,则()f x 在D 上为对数下(上) 凸函数的充要条件为对于任意x ∈D ,有2()()(())0(0)f x f x f x '''-≥≤先证下引理引理 2.1[2] (1) 若()g x 是[,]a b 上的下(上) 凸函数,则()()g x f x e = 为[,]a b e e 上的对数下(上) 凸函数.(2) 若()f x 是[,]c d 上的对数下(上) 凸函数,则()ln ()g x f x =为[ln ,ln ]c d 上的下(上) 凸数.证明(1) 任取12,[,]c d x x e e ∈,由()g x 在[,]c d 上是下凸函数,对任意01λ<<有()()121212[(1)]()(1)()121()()112[(1)][][]()()g x x g x g x g x g x f x x e e e e f x f x λλλλλλλλλλ+-+---+-=≤==(2)任取12,[ln ,ln ]x x c d ∈ ,由()f x 是[,]c d 上的对数下凸函数,对任意01λ<<有11212121212[(1)]ln [(1)]ln[()][()]ln ()(1)ln ()()(1)()g x x f x x f x f x f x f x g x g x λλλλλλλλλλ-+-=+-≤=+-=+-所以()g x 为区间[ln ,ln ]c d 上的下凸函数. (用类似方法可证上凸的情形)下证定理2.1[2] “⇐” 设[,]D c d =,()ln ()g x f x =,则 ()()[ln ()]()f xg x f x f x '''==,22()()[()]()()f x f x f x g x f x '''-''= 所以()g x 是为区间[ln ,ln ]c d 上的下凸函数,根据引理1 得()ln ()()g x f x e e f x ==为[ c ,d] 上的对数下凸函数“⇒” 若()f x 为[,]c d 上的对数下凸函数,由引理1 得()ln ()g x f x =为区间[ln ,ln ]c d 上的下凸函数,从而()0g x ''≥ ,对()ln ()g x f x =求二阶导数即得2()()(())0f x f x f x '''-≥. (用类似方法可证上凸的情形) .推论2.1[2] 设12(),()f x f x 是D 上的对数下(上) 凸函数,则1212()(),()()f x f x f x f x +也是D 上的对数下(上) 凸函数证明:设1212()()(),,,(0,1)g x f x f x x x D λ=+∀∈∈121122121111112221221121122212((1))((1))((1))()()()()[()()][()()]()()g x x f x x f x x f x f x f x fx f x f x f x f x g x g x λλλλλλλλλλλλλλ----+-=+-++-≤+≤+⨯+= 其中(A) 由..H older 不等式得到根据定义 2.2 得出1121()()f x f x +是D 上的对数下凸函数.122112[()()]()()()()f x f x f x f x f x f x '''=+12211212[()()]()()2()()()()f x f x f x f x f x f x f x f x ''''''''=++2121212222221111222[()()][()()]{[()()]}(){()()[()]}(){()()[()]}0f x f x f x f x f x f x f x f x f x f x f x f x f x f x '''-=''''''-+-≥根据定理2.1 得12(),()f x f x 是D 上的对数下凸函数. (用类似方法可证上凸的情形)用数学归纳法可将推论1 推广到有限情形.推论 2.2[2] 设()f x 是定义在D 上的正值函数,1) 若()f x 是对数下凸函数,则1()f x 在区间D 上是对数上凸函数. 2) 若()f x 是对数上凸函数,则1()f x 在区间D 上是对数下凸函数. 证明 1) 设1()()x f x φ=22322224241()()()2(())()(),()[]()()()()()2(())()()()(())()()[()][][][]()()()f x f x f x f x x x f x f x f x f x f x f x f x f x f x f x x x x f x f x f x φφφφφ''''-''''==-=-'''''''--'''-=--=-显然是小于0的,所以1()()x f x φ=是对数上凸函数,同理可证2) . 定理 2.2[2] (Jensen 型不等式) 设()f x 是D 上的正值对数下凸函数, 12,01, (1)i i n x D λλλλ∈<<+++=12112212(...)()()...()n n n n f x x x f x f x f x λλλλλλ+++≤ (*)若()f x 是D 上的正值对数上凸函数,则(*) 中不等号反向.证明 (用数学归纳法) 当2n =时,由定义2.2 知不等式(*) 成立. 假设n k =时不等式(*) 成立,即121122121(...)()()...()(1,0)kkk k k i i i f x x x f x f x f x λλλλλλλλ=+++≤=>∑ ,(1,2,...,1),i x D i k ∈=+设1(1,0)ki i i λλ==>∑111211121111221111111121111211[...()()]()()...()()()()...()()()k k k k k k k k k k k k k k k k k k k k k k k k k k k k k k x x f x x x x x f x f x f x f f x f x f x f x f x λλλλλλλλλλλλλλλλλλλλλλλλλλλ-+-+++--++++++-++-+++++++++≤+≤++ 所以当1n k =+时,不等式(*) 成立,从而对于一切自然数(2)n n ≥ 不等式(*) 成立. 用同样方法可证明上凸情形.当然这里的定理对凸函数也是成立的.在下面的运算性质中有介绍.也就是下面的Jensen 不等式 1,Jensen 不等式 2.引理 2.2[2] (凸函数的Hadamard 不等式) 设()x φ是区间D 上的下凸函数则对于任意,.a b D a b ∈≤有11()[()()]22b a a b x dx a b b aφφφφ+⎛⎫≤≤+ ⎪-⎝⎭⎰ (#) 若()x φ是区间D 上的上凸函数,则对于任意,.a b D a b ∈≤,(#)中不等号反向.定理 2.3[2] ( Hadamard 型不等式) 设():[,](0,)f x a b →+∞对数下凸函数,则11()()[()()]2ln ()ln ()b a a b f f x dx f b f a b a f a f b +≤≤---⎰ () 若():[,](0,)f x a b →+∞对数下凸函数,则(5) 中不等号反向.证明 由引理2.1 和引理2.2有1ln ()ln ()11ln ()()lim lim lim n f a bb f x n a a n i f a n n n b a f x dx e dx e n +∆→∞=+∆→∞→∞-==≥=∑⎰⎰nn 由平均值i=1(b-a )e (b-a )11(ln ())()2lim ()ln ()()()()2n i b ai f a b n n b a a n a b lmf b a e f x dxa b b a e b a f =-+∆-→∞+∑==-+≥-=-⎰1b-a (b-a)e (其中b a ∆=-)又令()ln ()x f x φ=,根据定义2.1,对于a x b <<,有()()()()()a b x b x a x b aφφφ-+-≤- ()()()()()()ln ()()()()()()()()()()()exp()|()()[]()()ln ()ln (b a x b a a b x b x a b b b b f x x b a a a a a b a a b b a a b b b b a b aa ab a f x dx edx e dx e dx b a b a e e dx e x b a b a b a b a e e b a f b f a φφφφφφφφφφφφφφφφφ-⎡⎤⎢⎥-⎣⎦-+------==≤--⎡⎤==⎢⎥--⎣⎦--=-=--⎰⎰⎰⎰⎰[()()])f b f a - 定理得证.2.3[3] 凸函数的性质 在讨论了一些对数凸函数的定理,引理,我们来看一看凸函数的运算性质以及它们实用的定理:(1) 若()f x 与()g x 均为区间[,]a b 上的凸函数,则()f x +()g x 也是区间[,]a b 上的凸函数.(2)若()f x 与()g x 为区间[,]a b 上的凸函数,则ⅰ)0λ≥,则()f x λ是[,]a b 上的凸函数;ⅱ)0λ<,则()f x λ是[,]a b 上的凹函数.(3) 设()f x 与()g x 都是[,]a b 上的非负单调递增的凸函数,则()()()h x f x g x =也是[,]a b 上的凸函数.证明:对任意12,x x ∈[,]a b 且12x x <和任意λ∈(0,1),因()f x 与()g x 在[,]a b 上单调递增,故 :1212[()()][()()]0f x f x g x g x --≥即: 12211122()()()()()()()()f x g x f x g x f x g x f x g x +≤+ (1) 又因为()f x 与()g x 在[,]a b 上的凸函数,故1212[(1)]()(1)()f x x f x f x λλλλ+-≤+-,2121g(x +(1-)x )g(x )+(1-)g(x )λλλλ≤ 而()0,()0f x g x ≥≥,设将上面两个不等式相乘,可得2122222211211[(1)][(1)]()()(1)[()()()()](1)()()f x x g x g x f x f x g x f x g x f x g x λλλλλλλλ+-+-≤+-++-又由⑴知21212222211211[(1)][(1)]()()(1)[()()()()(1)()()]f x x g x x g x f x f x g x f x g x f x g x λλλλλλλ+-+-≤+-++-=1122(1)()()()()f x g x f x g x λλ-+由凸函数的定义知:()()()h x f x g x =是[,]a b 上的凸函数.注:1°()f x 与()g x 非负不能少,2°(),()f x g x 单调递增不能少. (4)[4][5] 设()u ϕ是单调递增的凸函数,()u f x =是凸函数,则复合函数[()]f x ϕ也是凸函数.对于其他情况也有类似的情况的命题,如下列:我们也可以看一下单值有反函数的函数的反函数与自身的凸凹性的关系. 如下表:(5) 若()f x 为区间I 的凸函数,且()f x 不是常数,则()f x 在I 部不能达到最大值.2.4[3] 凸函数的等价定义和判定设函数f 在区间(,)a b 上有定义,则下列命题彼此互相等价:(1)对任意12,x x ∈(,)a b 及任意恒有1212[(1)]()(1)()f x x f x f x λλλλ+-≤+-(2)对任意i x ∈(,)a b 及任意i p >0. 1,2,...,i n =. 11n i i p -=∑ 恒有11()n ni i i i i i f p x p f x ==⎛⎫≤ ⎪⎝⎭∑∑ (3)对任意1,2,(,)x x x a b ∈, 12x x x <<,恒有12121212()()()()()()f x f x f x f x f x f x x x x x x x---≤≤--- (4)在(,)a b 上曲线在其每一点处具有不垂直于x 轴的左、右切线,并且曲线在左、右切线之上.(5)若在(,)a b 存在单调递增的函数()x ϕ.以及0x ∈(,)a b ,使得对任意(,)x a b ∈,恒有00()()()xx f x f x t dt ϕ-=⎰,(6)对任意12,x x ∈(,)a b ,12x x <,恒有21121221()()1()22x x x x f x f x f f t dt x x ++⎛⎫≤≤ ⎪-⎝⎭⎰ (7)对任意12,(,)x x a b ∈,恒有1212()()22x x f x f x f ++⎛⎫≤ ⎪⎝⎭对于凸函数定义等价性的证明,可参看[4]及[5].对于等价定义(5)事实上,我们也有类似的这样一个定理: 定理 2.4 设函数f 在[,]a b 上连续,在(,)a b 上可导,则f 在[,]a b 上为上(下)凸函数(严格上(下)凸函数)的一个必要充分条件f '是在(,)a b 上递增(减)(严格递增(减)).证明 先证条件是必要的.设()12,(,)x x a b ⊂.只要x x '与满足12x x x x '<<<,由于等价定义(3)可知12121212()()()()()()f x f x f x f x f x f x x x x x x x '---≤≤'--- 在上式中令12,x x x x +-'→→,得211221()()()()f x f x f x f x x x -''≤≤-. 在是严格上凸函数的情形,我们取一点*x 满足*12x x x <<,从而得出**1212**12()()()()()()f x f x f x f x f x f x x x x x--''≤<≤--. 这样就得出了严格的不等式12()()f x f x ''<,必要性得证.再证充分性.设f '是在(,)a b 上递增.对任何()12,x x x ∈,由Lagrange 中值定理,可只存在()12,x x ξ∈与()12,x x η∈,使得11()()()f x f x f x x ξ-'=-,22()()()f x f x f x x η-'=- 因为x ξη<<,所以()()f f ξη''≤.从而有1212()()()()f x f x f x f x x x x x--≤--所以,可知函数f 在[,]a b 上为上凸函数.容易看出,当f '严格递增时,()()f f ξη''<.上述不等式中成立着严格的不等号,从而函数f 在[,]a b 上是严格的上凸函数.同理可以证明下凸时的情景.当函数f 在[,]a b 有二阶导数时,我们有下列应用起来就会更方便的定理 定理 2.5 设函数f 在[,]a b 上连续,f 在(,)a b 有二阶导数,则f 在[,]a b 上为上凸函数(下凸函数)的充分条件0(0)f f ''''≥≤在(,)a b 成立;而f 在[,]a b 上为严格上(下)凸函数的充分必要条件是0(0)f f ''''≥≤在(,)a b 成立并且在(,)a b 的任何开的子区间f ''不恒等于0.证明 第一个结论,由于0f ''≥得出f '在(,)a b 上递增再由定理4可得出.同理可证明下凸时的情景; 第二个结论,先证充分性 由于0f ''≥在(,)a b 成立并且在(,)a b 的任何开的子区间f ''不恒等于0.对任意12,(,)x x a b ∈,12x x <,又由于2121()()()x x f x f x f x dx ''''=+⎰,所以21()()f x f x ''>.所以函数f 在[,]a b 上为严格的凸函数.充分性得证.再证必要性(反证法) 因为函数f 在[,]a b 上为严格凸函数,对任意12,(,)x x a b ∈,12x x <,则21()()f x f x ''>,而由于2121()()()x x f x f x f x dx ''''=+⎰,若是有一个(,)a b 的子区间恒等于0.不妨设为(,)(,)a b ξη⊂,对任意(,)x ξη∈,()0f x ''=.则由于21()()()x x f f f x dx ηξ''''=+⎰,()()f f ξη''=,这与已知条件相矛盾.所以,必要性得证.同理可证明下凸时的情景. 所以,定理得证.关于凸函数的判定有很多,应用围最广的是Jensen 不等式. Jensen 不等式 1 设()f x 在区间I 上有定义,()f x 为凸函数,当且仅当12,,...,n x x x I ∀∈1212...()()...()n n x x x f x f x f x f n n ++++++⎛⎫≤⎪⎝⎭(J1) 此外,当且仅当12...n x x x === 时,上式等号成立(证明略请参考附[1]). Jensen 不等式 2 12,,...,[,]n x x x a b ∀∈,12,,...,0n λλλ>,且11ni i λ==∑,1.则()f x 为凸函数的充要条件为:11()()n ni i i i i i f x f x λλ==≤∑∑ (J2)此外,上式当且仅当12...n x x x === 时,等号成立.(证明略请参考附[1]). 这里对任意12,,...,0n βββ>,若是令1ii nii βλβ==∑,那么就有1111()nni i i i i i n n i i i i x f x f ββββ====⎛⎫ ⎪ ⎪≤⎪ ⎪⎝⎭∑∑∑∑ (J3) 每个凸函数都有一个Jensen 不等式,Jensen 不等式的应用围甚广,既可用于求解不等式问题,又可用于证明不等式定理,应用Jensen 不等式解题的关键有两条:一是必须先判明函数的上(下)凸性,二是直接应用Jensen 不等式有困难时,可以根据命题的特点,选择恰当的上凸函数和下凸函数,然后再进行解答.3 凸函数以及对数凸函数的应用在许多证明题中,我们常常遇到一些不等式的证明,其中有一类不等式利用凸函数的性质来证明可以非常简洁、巧妙.证明不等式是凸函数的一个重要应用领域,但关键是构造能够解决问题的凸函数.例 1[1] 利用凸函数证明调和平均值H ≤几何平均值G ≤对数平均值L ≤指数平均值E ≤算术平均值A.证明:事实上,我们可以用凸函数理论证明,对任意0(1,2,...,)ix i n = 有1212 (111)...nnx x x n nx x x +++≤≤+++ (2)只要将不等式各部分同时取对数,这时左边的不等式可变为121111...1111ln (ln ln ...ln )n nx x x n n x x x +++-≤----.从而由函数()ln f x x =-在(0,)+∞上的(严格)凸性可得;右边的不等式可直接由()ln g x x =上的(0,)+∞(严格)下凸性可得.(具体证明可参看[2])为了证明例1 中的连不等式,我们先来看下面两个小题:(1) 设0(1,2,...,)i a i n >=且不全相等,0(1,2,...,)i p i n >=有不等式链11111ln ln exp exp n n nii i i i i i i i i nn n ii i i i n i i p a p a p a a p p p a ======⎛⎫⎛⎫ ⎪ ⎪ ⎪≤≤ ⎪ ⎪ ⎪ ⎪⎪⎝⎭⎝⎭∑∑∑∑∑∑ (3) 证:凸函数()ln f x x =-的Jensen 不等式:取0i q >,11ni i q ==∑,0(1,2,...,).i a i n >=得11ln ln n n i i i i i i q a q a ==-≤-∑∑ [4] 111ln ln nn i i i i i i q q a a ==-≤-∑∑ (5)在[4]中令1i ini ii ip a q p a ==∑得 1111exp ln nn niiii ni i i i iii ip p p a p a a a ====⎛⎫≤ ⎪⎝⎭∑∑∑∑ (6)又由(4),(5)可得 1111in nq i i i n i i i i ia q a q a ===≤≤∑∏∑ (7)在此令1ini i i p q p ==∑,可得111111ln exp nn ni i i i ii i i n n n ii i i i i i p p a p a p p p a ======⎛⎫ ⎪≤≤ ⎪ ⎪ ⎪⎝⎭∑∑∑∑∑∑ (8) 联立(6),(8)既得证 (3).(2) 设()()f x p x 与在[,]a b 上正的连续函数且()f x ≠常数,在⑻中作代换i b a p p a i n -⎛⎫=+ ⎪⎝⎭,i b a a f a i n -⎛⎫=+ ⎪⎝⎭并在“∑”号后均乘b a n -,由0b a ->,不改变原不等号方向.令n →∞ 便得(3)的积分形式:ln ln exp exp b bb ba aa ab b bba aa ap fdx pdxp fdx pfdx f p p pdx pdxdx dx f f ⎛⎫⎛⎫ ⎪⎪ ⎪≤≤≤ ⎪ ⎪ ⎪ ⎪⎝⎭⎝⎭⎰⎰⎰⎰⎰⎰⎰⎰(3)'在(3)'中令()1,()p x f x x ==()11ln ln ln ln 2b ab a b a b ab a e ----+⎛⎫≤≤≤⎪-⎝⎭再联立(2),得出H G L E A ≤≤≤≤.例 2 (1)在锐角ABC ∆中,证明1cos cos cos 2A B C ++≤, (2)12,,...,n a a a 设为正数,证明恒成立12...n a a a n +++≥. 证明 (1)令()cos()f x x =-,(0,)x π∈.由于()cos()0f x x ''=>,(0,)2x π∈.所以()f x 在(0,)2x π∈上凸函数,所以由于(J1)()()()()33f A f B f C A B Cf ++++≥,即cos()cos()cos()s()33A B C A B C co ---++≥-1()2=-即1cos cos cos 2A B C ++≤;(2) 令()ln ,(0,)g x x x =-∈+∞,所以21()0,(0,)g x x x''=>∈+∞, 故()g x 是在(0,)+∞上的上凸函数.也是根据(J1)121212121212()()...()...()ln ln ...ln ...ln()ln ln ...ln ...ln()n nn nn n g a g a g a a a a g n n a a a a a a n na a a a a a n n++++++≥++++++-≥-++++++≤即即从而,有12...n a a a n+++≥.下面我们再看一个用对数凸函数证明的不等式题. 例 3[2]10,0,12ni i i πλλ=<<>=∑i 设x ,则12112212sin(...)sin sin ...sin n n n n x x x x x x λλλλλλ+++≥ (&)12112212cos(...)cos cos ...cos n n n n x x x x x x λλλλλλ+++≥(%)证明 设()sin()f x x =,由于2()()[()]10f x f x f x '''-=-<,故sin()x 是(0,)2π上的对数凸函数,同理cos()x 也是(0,)2π上对数凸函数.根据定理2即可得(&),(%).例 4 设()f x 在[,]a b 上可积,且()m f x M ≤≤,()t ϕ是在[,]m M 上的连续下凸函数,则11()(())b b a a f x dx f x dx b a b a ϕϕ⎛⎫≥ ⎪--⎝⎭⎰⎰. 证明 令,()k n k f f a b a n ⎛⎫=+- ⎪⎝⎭,,1()k n x b a n ∆=-.由于()t ϕ是凸函数,故有1,2,,1,2,,...()()...()n n n n n n n n f f f f f f n n ϕϕϕϕ++++++⎛⎫≥⎪⎝⎭. 由定积分的定义,上式就相当于,,,,11()n ni n i n i n i ni i f f b a b a ϕϕ==⎛⎫∆∆ ⎪ ⎪≥-- ⎪⎪⎝⎭∑∑,,1()k n x b a n ∆=- 在上式中令n →∞时, 则有11()(())b b a a f x dx f x dx b a b a ϕϕ⎛⎫≥⎪--⎝⎭⎰⎰. 命题得证.例 5[7]设,i i a b R +∈,111,2,...,,,n n i i i i i n a b ====∑∑则21112nni i i i i ia a ab ==≥+∑∑.证明 记1ni i s a ==∑,11ni i a s ==∑,将21112nni i i i i i a a a b ==≥+∑∑变为11121n ii i ia b s a =≥+∑,那么取11i ib a +作为函数1()1f x x =+,则由于3()2(1)0f x x -''=+>,再令i i i b x a =,i i a sλ=所以根据凸函数性质和(J3)得出11111211ni n i i i i i i a b s x a λ==≥=++∑∑结论本文主要讨论了凸函数以及对数凸函数一类重要的函数的概念,包括它们的一些定义,性质,定理,引理和它们在证明一些不等式的重要应用.本文介绍了Jensen不等式,Hadamard不等式,叙述了一些定理,引理,性质并给出了它们的证明,并指出它们在判断凸函数的应用.本文还试就凸函数的等价定义、性质和在证明不等式中的应用等问题作一初步的探讨,以便进一步了解凸函数的性质及其在证明不等式时的作用.最后举出了一些例题来具体的来体现凸函数以及对数凸函数在不等式证明的应用.参考文献:[1]汪文珑.数学分析选讲[M].文理学院数学系,2001[2] 琼.对数凸函数的Jensen型和Hadamard型不等式[J].学报,,2005,3[3]查良凇.凸函数及其在不等式证明中的应用[J].工贸职业技术学院学报,,2005,3[4]燕建梁,喜善.凸函数的性质及其在不等式证明中的应用[J].教育学院学报,,2002,4[5]T.M菲赫金哥尔茨普.微积分教程[M].1965: 290-300[6]常庚哲,史济怀.数学分析教程(上册)(M).高等教育,2003:167-176[7]碧荣.凸函数及其性质在不等式证明中的应用[J].广西师学院学报,,2004,2[8]白景华.图函数的性质、等价定义及应用[J].大学学报,,2003,2[9]Satish Shirali, Harkrishan L. Vasudeva. Mathematical analysis[M]. Alpha Science International Ltd., c2006.[10]Tom M. Apostol.Mathematical analysis[M].China Machine Press, 2004.致这是本人的第一篇论文,所以在多方面没有指导老师金洪老师的指导是很难进行下去的.老师从我的选题开始便给予了很大帮助,在以后的开题,开题报告,初稿的资料搜索,初稿出来后的校正,进一步的改进都给予了极大帮助,使我在论文的完成进程中得以较为平坦地进行下去.在论文的写作的进行中,我同组等同学也给了我很多帮助.在此表示感.也在此对我们的学校师大学以及我校资料室提供这样一个学习环境和帮助,表示感.也感那在身后的帮助.。
