土木工程外文翻译-原文
土木工程毕业设计外文翻译原文+翻译

The bridge crack produced the reason to simply analyseIn recent years, the traffic capital construction of our province gets swift and violent development, all parts have built a large number of concrete bridges. In the course of building and using in the bridge, relevant to influence project quality lead of common occurrence report that bridge collapse even because the crack appears The concrete can be said to " often have illness coming on " while fracturing and " frequently-occurring disease ", often perplex bridge engineers and technicians. In fact , if take certain design and construction measure, a lot of cracks can be overcome and controlled. For strengthen understanding of concrete bridge crack further, is it prevent project from endanger larger crack to try one's best, this text make an more overall analysis , summary to concrete kind and reason of production , bridge of crack as much as possible, in order to design , construct and find out the feasible method which control the crack , get the result of taking precautions against Yu WeiRan.Concrete bridge crack kind, origin cause of formation In fact, the origin cause of formation of the concrete structure crack is complicated and various, even many kinds of factors influence each other , but every crack has its one or several kinds of main reasons produced . The kind of the concrete bridge crack, on its reason to produce, can roughly divide several kinds as follows :(1) load the crack caused Concrete in routine quiet .Is it load to move and crack that produce claim to load the crack under the times of stress bridge, summing up has direct stress cracks , two kinds stress crack onces mainly. Direct stress crack refer to outside load direct crack that stress produce that cause. The reason why the crack produces is as follows, 1, Design the stage of calculating , does not calculate or leaks and calculates partly while calculating in structure; Calculate the model is unreasonable; The structure is supposed and accorded with by strength actually by strength ; Load and calculate or leak and calculate few; Internal force and matching the mistake in computation of muscle; Safety coefficient of structure is not enough. Do not consider the possibility that construct at the time of the structural design; It is insufficientto design the section; It is simply little and assigning the mistake for reinforcing bar to set up; Structure rigidity is insufficient; Construct and deal with improperly; The design drawing can not be explained clearly etc.. 2, Construction stage, does not pile up and construct the machines , material limiting ; Is it prefabricate structure structure receive strength characteristic , stand up , is it hang , transport , install to get up at will to understand; Construct not according to the design drawing, alter the construction order of the structure without authorization , change the structure and receive the strength mode; Do not do the tired intensity checking computations under machine vibration and wait to the structure . 3, Using stage, the heavy-duty vehicle which goes beyond the design load passes the bridge; Receive the contact , striking of the vehicle , shipping; Strong wind , heavy snow , earthquake happen , explode etc.. Stress crack once means the stress of secondary caused by loading outside produces the crack. The reason why the crack produces is as follows, 1, In design outside load function , because actual working state and routine , structure of thing calculate have discrepancy or is it consider to calculate, thus cause stress once to cause the structure to fracture in some position. Two is it join bridge arch foot is it is it assign " X " shape reinforcing bar , cut down this place way , section of size design and cut with scissors at the same time to adopt often to design to cut with scissors, theory calculate place this can store curved square in , but reality should is it can resist curved still to cut with scissors, so that present the crack and cause the reinforcing bar corrosion. 2, Bridge structure is it dig trough , turn on hole , set up ox leg ,etc. to need often, difficult to use a accurate one diagrammatic to is it is it calculate to imitate to go on in calculating in routine, set up and receive the strength reinforcing bar in general foundation experience. Studies have shown , after being dug the hole by the strength component , it will produce the diffraction phenomenon that strength flows, intensive near the hole in a utensil, produced the enormous stress to concentrate. In long to step prestressing force of the continuous roof beam , often block the steel bunch according to the needs of section internal force in stepping, set up the anchor head, but can often see the crack in the anchor firm section adjacent place. So if deal with improper, in corner or component form sudden change office , block place to be easy to appear crack strengthreinforcing bar of structure the. In the actual project, stress crack once produced the most common reason which loads the crack. Stress crack once belong to one more piece of nature of drawing , splitting off , shearing. Stress crack once is loaded and caused, only seldom calculate according to the routine too, but with modern to calculate constant perfection of means, times of stress crack to can accomplish reasonable checking computations too. For example to such stresses 2 times of producing as prestressing force , creeping ,etc., department's finite element procedure calculates levels pole correctly now, but more difficult 40 years ago. In the design, should pay attention to avoiding structure sudden change (or section sudden change), when it is unable to avoid , should do part deal with , corner for instance, make round horn , sudden change office make into the gradation zone transition, is it is it mix muscle to construct to strengthen at the same time, corner mix again oblique to reinforcing bar , as to large hole in a utensil can set up protecting in the perimeter at the terms of having angle steel. Load the crack characteristic in accordance with loading differently and presenting different characteristics differently. The crack appear person who draw more, the cutting area or the serious position of vibration. Must point out , is it get up cover or have along keep into short crack of direction to appear person who press, often the structure reaches the sign of bearing the weight of strength limit, it is an omen that the structure is destroyed, its reason is often that sectional size is partial and small. Receive the strength way differently according to the structure, the crack characteristic produced is as follows: 1, The centre is drawn. The crack runs through the component cross section , the interval is equal on the whole , and is perpendicular to receiving the strength direction. While adopting the whorl reinforcing bar , lie in the second-class crack near the reinforcing bar between the cracks. 2, The centre is pressed. It is parallel on the short and dense parallel crack which receive the strength direction to appear along the component. 3, Receive curved. Most near the large section from border is it appear and draw into direction vertical crack to begin person who draw curved square, and develop toward neutralization axle gradually. While adopting the whorl reinforcing bar , can see shorter second-class crack among the cracks. When the structure matches muscles less, there are few but wide cracks, fragility destruction may take place in thestructure 4, Pressed big and partial. Heavy to press and mix person who draw muscle a less one light to pigeonhole into the component while being partial while being partial, similar to receiving the curved component. 5, Pressed small and partial. Small to press and mix person who draw muscle a more one heavy to pigeonhole into the component while being partial while being partial, similar to the centre and pressed the component. 6, Cut. Press obliquly when the hoop muscle is too dense and destroy, the oblique crack which is greater than 45?? direction appears along the belly of roof beam end; Is it is it is it destroy to press to cut to happen when the hoop muscle is proper, underpart is it invite 45?? direction parallel oblique crack each other to appear along roof beam end. 7, Sprained. Component one side belly appear many direction oblique crack, 45?? of treaty, first, and to launch with spiral direction being adjoint. 8, Washed and cut. 4 side is it invite 45?? direction inclined plane draw and split to take place along column cap board, form the tangent plane of washing. 9, Some and is pressed. Some to appear person who press direction roughly parallel large short cracks with pressure.(2) crack caused in temperature changeThe concrete has nature of expanding with heat and contract with cold, look on as the external environment condition or the structure temperature changes, concrete take place out of shape, if out of shape to restrain from, produce the stress in the structure, produce the temperature crack promptly when exceeding concrete tensile strength in stress. In some being heavy to step foot-path among the bridge , temperature stress can is it go beyond living year stress even to reach. The temperature crack distinguishes the main characteristic of other cracks will be varied with temperature and expanded or closed up. The main factor is as follows, to cause temperature and change 1, Annual difference in temperature. Temperature is changing constantly in four seasons in one year, but change relatively slowly, the impact on structure of the bridge is mainly the vertical displacement which causes the bridge, can prop up seat move or set up flexible mound ,etc. not to construct measure coordinate , through bridge floor expansion joint generally, can cause temperature crack only when the displacement of the structure is limited, for example arched bridge , just bridge etc. The annual difference in temperature of our country generally changes therange with the conduct of the average temperature in the moon of January and July. Considering the creep characteristic of the concrete, the elastic mould amount of concrete should be considered rolling over and reducing when the internal force of the annual difference in temperature is calculated. 2, Rizhao. After being tanned by the sun by the sun to the side of bridge panel , the girder or the pier, temperature is obviously higher than other position, the temperature gradient is presented and distributed by the line shape . Because of restrain oneself function, cause part draw stress to be relatively heavy, the crack appears. Rizhao and following to is it cause structure common reason most , temperature of crack to lower the temperature suddenly 3, Lower the temperature suddenly. Fall heavy rain , cold air attack , sunset ,etc. can cause structure surface temperature suddenly dropped suddenly, but because inside temperature change relatively slow producing temperature gradient. Rizhao and lower the temperature internal force can adopt design specification or consult real bridge materials go on when calculating suddenly, concrete elastic mould amount does not consider converting into and reducing 4, Heat of hydration. Appear in the course of constructing, the large volume concrete (thickness exceeds 2. 0), after building because cement water send out heat, cause inside very much high temperature, the internal and external difference in temperature is too large, cause the surface to appear in the crack. Should according to actual conditions in constructing, is it choose heat of hydration low cement variety to try one's best, limit cement unit's consumption, reduce the aggregate and enter the temperature of the mould , reduce the internal and external difference in temperature, and lower the temperature slowly , can adopt the circulation cooling system to carry on the inside to dispel the heat in case of necessity, or adopt the thin layer and build it in succession in order to accelerate dispelling the heat. 5, The construction measure is improper at the time of steam maintenance or the winter construction , the concrete is sudden and cold and sudden and hot, internal and external temperature is uneven , apt to appear in the crack. 6, Prefabricate T roof beam horizontal baffle when the installation , prop up seat bury stencil plate with transfer flat stencil plate when welding in advance, if weld measure to be improper, iron pieces of nearby concrete easy to is it fracture to burn. Adopt electric heat piece draw law piece draw prestressing force at the component ,prestressing force steel temperature can rise to 350 degrees Centigrade , the concrete component is apt to fracture. Experimental study indicates , are caused the intensity of concrete that the high temperature burns to obviously reduce with rising of temperature by such reasons as the fire ,etc., glueing forming the decline thereupon of strength of reinforcing bar and concrete, tensile strength drop by 50% after concrete temperature reaches 300 degrees Centigrade, compression strength drops by 60%, glueing the strength of forming to drop by 80% of only round reinforcing bar and concrete; Because heat, concrete body dissociate ink evaporate and can produce and shrink sharply in a large amount(3) shrink the crack causedIn the actual project, it is the most common because concrete shrinks the crack caused. Shrink kind in concrete, plasticity shrink is it it shrinks (is it contract to do ) to be the main reason that the volume of concrete out of shape happens to shrink, shrink spontaneously in addition and the char shrink. Plasticity shrink. About 4 hours after it is built that in the course of constructing , concrete happens, the cement water response is fierce at this moment, the strand takes shape gradually, secrete water and moisture to evaporate sharply, the concrete desiccates and shrinks, it is at the same time conduct oneself with dignity not sinking because aggregate,so when harden concrete yet,it call plasticity shrink. The plasticity shrink producing amount grade is very big, can be up to about 1%. If stopped by the reinforcing bar while the aggregate sinks, form the crack along the reinforcing bar direction. If web , roof beam of T and roof beam of case and carry baseplate hand over office in component vertical to become sectional place, because sink too really to superficial obeying the web direction crack will happen evenly before hardenning. For reducing concrete plasticity shrink,it should control by water dust when being construct than,last long-time mixing, unloading should not too quick, is it is it take closely knit to smash to shake, vertical to become sectional place should divide layer build. Shrink and shrink (do and contract). After the concrete is formed hard , as the top layer moisture is evaporated progressively , the humidity is reduced progressively , the volume of concrete is reduced, is called and shrunk to shrink (do and contract). Because concrete top layermoisture loss soon, it is slow for inside to lose, produce surface shrink heavy , inside shrink a light one even to shrink, it is out of shape to restrain from by the inside concrete for surface to shrink, cause the surface concrete to bear pulling force, when the surface concrete bears pulling force to exceed its tensile strength, produce and shrink the crack. The concrete hardens after-contraction to just shrink and shrink mainly .Such as mix muscle rate heavy component (exceed 3% ), between reinforcing bar and more obvious restraints relatively that concrete shrink, the concrete surface is apt to appear in the full of cracks crackle. Shrink spontaneously. Spontaneous to it shrinks to be concrete in the course of hardenning , cement and water take place ink react, the shrink with have nothing to do by external humidity, and can positive (whether shrink, such as ordinary portland cement concrete), can negative too (whether expand, such as concrete, concrete of slag cement and cement of fly ash). The char shrinks. Between carbon dioxide and hyrate of cement of atmosphere take place out of shape shrink that chemical reaction cause. The char shrinks and could happen only about 50% of humidity, and accelerate with increase of the density of the carbon dioxide. The char shrinks and seldom calculates . The characteristic that the concrete shrinks the crack is that the majority belongs to the surface crack, the crack is relatively detailed in width , and criss-cross, become the full of cracks form , the form does not have any law . Studies have shown , influence concrete shrink main factor of crack as follows, 1, Variety of cement , grade and consumption. Slag cement , quick-hardening cement , low-heat cement concrete contractivity are relatively high, ordinary cement , volcanic ash cement , alumina cement concrete contractivity are relatively low. Cement grade low in addition, unit volume consumption heavy rubing detailed degree heavy, then the concrete shrinks the more greatly, and shrink time is the longer. For example, in order to improve the intensity of the concrete , often adopt and increase the cement consumption method by force while constructing, the result shrinks the stress to obviously strengthen . 2, Variety of aggregate. Such absorbing water rates as the quartz , limestone , cloud rock , granite , feldspar ,etc. are smaller, contractivity is relatively low in the aggregate; And such absorbing water rates as the sandstone , slate , angle amphibolite ,etc. are greater, contractivity is relatively high. Aggregate grains of foot-path heavy to shrink light inaddition, water content big to shrink the larger. 3, Water gray than. The heavier water consumption is, the higher water and dust are, the concrete shrinks the more greatly. 4, Mix the pharmaceutical outside. It is the better to mix pharmaceutical water-retaining property outside, then the concrete shrinks the smaller. 5, Maintain the method . Water that good maintenance can accelerate the concrete reacts, obtain the intensity of higher concrete. Keep humidity high , low maintaining time to be the longer temperature when maintaining, then the concrete shrinks the smaller. Steam maintain way than maintain way concrete is it take light to shrink naturall. 6, External environment. The humidity is little, the air drying , temperature are high, the wind speed is large in the atmosphere, then the concrete moisture is evaporated fast, the concrete shrinks the faster. 7, Shake and smash the way and time. Machinery shake way of smashing than make firm by ramming or tamping way concrete contractivity take little by hand. Shaking should determine according to mechanical performance to smash time , are generally suitable for 55s / time. It is too short, shake and can not smash closely knit , it is insufficient or not even in intensity to form the concrete; It is too long, cause and divide storey, thick aggregate sinks to the ground floor, the upper strata that the detailed aggregate stays, the intensity is not even , the upper strata incident shrink the crack. And shrink the crack caused to temperature, worthy of constructing the reinforcing bar againing can obviously improve the resisting the splitting of concrete , structure of especially thin wall (thick 200cm of wall ). Mix muscle should is it adopt light diameter reinforcing bar (8 |? construct 14 |? ) to have priority , little interval assign (whether @ 10 construct @ 15cm ) on constructing, the whole section is it mix muscle to be rate unsuitable to be lower than 0 to construct. 3%, can generally adopt 0 . 3%~0. 5%.(4), crack that causes out of shape of plinth of the groundBecause foundation vertical to even to subside or horizontal direction displacement, make the structure produce the additional stress, go beyond resisting the ability of drawing of concrete structure, cause the structure to fracture. The even main reason that subside of the foundation is as follows, 1, Reconnoitres the precision and is not enough for , test the materials inaccuratly in geology. Designing, constructing without fully grasping the geological situation, this is the main reason that cause the ground not to subside evenly .Such as hills area or bridge, district of mountain ridge,, hole interval to be too far when reconnoitring, and ground rise and fall big the rock, reconnoitring the report can't fully reflect the real geological situation . 2, The geological difference of the ground is too large. Building it in the bridge of the valley of the ditch of mountain area, geology of the stream place and place on the hillside change larger, even there are weak grounds in the stream, because the soil of the ground does not causes and does not subside evenly with the compressing. 3, The structure loads the difference too big. Under the unanimous terms, when every foundation too heavy to load difference in geological situation, may cause evenly to subside, for example high to fill out soil case shape in the middle part of the culvert than to is it take heavy to load both sides, to subside soon heavy than both sides middle part, case is it might fracture to contain 4, The difference of basic type of structure is great. Unite it in the bridge the samly , mix and use and does not expand the foundation and a foundation with the foundation, or adopt a foundation when a foot-path or a long difference is great at the same time , or adopt the foundation of expanding when basis elevation is widely different at the same time , may cause the ground not to subside evenly too 5, Foundation built by stages. In the newly-built bridge near the foundation of original bridge, if the half a bridge about expressway built by stages, the newly-built bridge loads or the foundation causes the soil of the ground to consolidate again while dealing with, may cause and subside the foundation of original bridge greatly 6, The ground is frozen bloatedly. The ground soil of higher moisture content on terms that lower than zero degree expands because of being icy; Once temperature goes up , the frozen soil is melted, the setting of ground. So the ground is icy or melts causes and does not subside evenly . 7, Bridge foundation put on body, cave with stalactites and stalagmites, activity fault,etc. of coming down at the bad geology, may cause and does not subside evenly . 8, After the bridge is built up , the condition change of original ground . After most natural grounds and artificial grounds are soaked with water, especially usually fill out such soil of special ground as the soil , loess , expanding in the land ,etc., soil body intensity meet water drop, compress out of shape to strengthen. In the soft soil ground , season causes the water table to drop to draw water or arid artificially, the ground soil layer consolidates and sinks again,reduce the buoyancy on the foundation at the same time , shouldering the obstruction of rubing to increase, the foundation is carried on one's shoulder or back and strengthened .Some bridge foundation is it put too shallow to bury, erode , is it dig to wash flood, the foundation might be moved. Ground load change of terms, bridge nearby is it is it abolish square , grit ,etc. in a large amount to put to pile with cave in , landslide ,etc. reason for instance, it is out of shape that the bridge location range soil layer may be compressed again. So, the condition of original ground change while using may cause and does not subside evenly Produce the structure thing of horizontal thrust to arched bridge ,etc., it is the main reason that horizontal displacement crack emerges to destroy the original geological condition when to that it is unreasonable to grasp incompletely , design and construct in the geological situation.桥梁裂缝产生原因浅析近年来,我省交通基础建设得到迅猛发展,各地建立了大量的混凝土桥梁。
(完整)土木工程外文翻译

原文Prestressed ConcreteConcrete is strong in compression, but weak in tension: Its tensile strength varies from 8 to 14 percent of its compressive strength。
Due to such a low tensile capacity, flexural cracks develop at early stages of loading. In order to reduce or prevent such cracks from developing, a concentric or eccentric force is imposed in the longitudinal direction of the structural element. This force prevents the cracks from developing by eliminating or considerably reducing the tensile stresses at the critical midspan and support sections at service load, thereby raising the bending, shear, and torsional capacities of the sections。
The sections are then able to behave elastically, and almost the full capacity of the concrete in compression can be efficiently utilized across the entire depth of the concrete sections when all loads act on the structure.Such an imposed longitudinal force is called a prestressing force, i.e., a compressive force that prestresses the sections along the span of the structural element prior to the application of the transverse gravity dead and live loads or transient horizontal live loads。
土木工程专业英语课文翻译考试必备

土木工程专业英语课文_翻译_考试必备土木工程专业英语课文翻译The principal construction materials of earlier times were wood and masonry brick, stone, or tile, and similar materials. The courses or layers were bound together with mortar or bitumen, a tar like substance, or some other binding agent. The Greeks and Romanssometimes used iron rods or claps to strengthen their building. The columns of the Parthenon in Athens, for example, have holes drilled in them for iron bars that have now rusted away. The Romans also used a natural cement called puzzling, made from volcanic ash, that became as hard as stone under water.早期时代的主要施工材料,木材和砌体砖,石,或瓷砖,和类似的材料;这些课程或层密切联系在一起,用砂浆或沥青,焦油一个样物质,或其他一些有约束力的代理人;希腊人和罗马人有时用铁棍或拍手以加强其建设;在雅典的帕台农神庙列,例如,在他们的铁钻的酒吧现在已经生锈了孔;罗马人还使用了天然水泥称为令人费解的,由火山灰制成,变得像石头一样坚硬在水中;Both steel and cement, the two most important construction materials of modern times, were introduced in the nineteenth century. Steel, basically an alloy of iron and a small amount of carbon had been made up to that time by a laborious process that restricted it to such special uses as sword blades. After the invention of the Bessemer process in 1856, steel was available in large quantities at low prices. The enormous advantage of steel is its tensile force which, as we have seen, tends to pull apart many materials. New alloys have further, which is a tendency for it to weaken as a result of continual changes in stress.钢铁和水泥,两个最重要的现代建筑材料,介绍了在十九世纪;钢,铁,基本上是少量的碳合金已作出了这一由一个艰苦的过程,限制它的刀刃等特殊用途的时间;后在1856年发明贝塞麦过程,钢在低价格大批量供货;钢铁的巨大优势是它的拉伸力,正如我们所看到的,往往会拉开许多材料;新合金进一步,这是一个趋势,它削弱了在压力不断变化的结果;Modern cement, called Portland cement, was invented in 1824. It is a mixture of limestone and clay, which is heated and then ground into a power. It is mixed at or near the construction site with sand, aggregate small stones, crushed rock, or gravel, and water to make concrete. Different proportions of the ingredients produce concrete with different strength and weight. Concrete is very versatile; it can be poured, pumped, or even sprayed into all kinds of shapes. And whereas steel has great tensile strength, concrete has great strength under compression. Thus, the two substances complement each other. 现代水泥,称为硅酸盐水泥,发明于1824年;它是石灰石和粘土的混合物,被加热,然后进入电源地;它是混合达到或接近沙施工现场,聚集的小石头,碎石,或石子和水,使混凝土;不同比例的成分产生不同强度和重量混凝土;混凝土是非常灵活,它可浇,泵浦,或连成各种形状喷洒;和鉴于钢具有很大的拉伸强度,混凝土受压的伟大力量;因此,这两种物质是相辅相成的;They also complement each other in another way: they have almost the same rate of contraction and expansion. They therefore can work together in situations where both compression and tension are factors. Steel rods are embedded in concrete to make reinforced concrete in concrete beams or structures where tensions will develop. Concrete and steel also form such a strong bond the force that unites them that the steel cannot slip within theconcrete. Still another advantage is that steel does not rust in concrete. Acid corrodessteel, whereas concrete has an alkaline chemical reaction, the oppositeof acid.他们还以另一种方式补充对方:他们几乎在同样的速度收缩和扩张;因此,他们可以一起工作的情况下压缩和紧张的因素;钢条是嵌在混凝土,使钢筋混凝土结构中混凝土梁或地方的紧张局势会发展;混凝土和钢也形成如此强烈的纽带团结的力量他们的钢材,不滑内的混凝土;还有一个好处是,不生锈的钢混凝土;酸腐蚀钢,而混凝土的碱性化学反应,酸相反;The adoption of structural steel and reinforced concrete caused major changes in traditional construction practices. It was no longer necessary to use thick walls of stone or brick for multistory buildings, and it became much simpler to build fire-resistant floors. Both these changes served to reduce the cost of construction. It also became possible to erect buildings with greater heights and longer spans.结构钢和钢筋混凝土建筑采用传统的做法造成了重大变化;它不再需要使用的石块或砖头厚的多层建筑物的墙壁,成为更简单,建立防火地板;这些变化都有助于降低建设成本;它也成为可能有更大的直立高度和时间跨度的建筑;Since the weight of modern structures is carried by the steel or concrete frame, the walls do not support the building. They have become curtain walls, which keep out the weather and let in light. In the earlier steel orconcrete frame building, the curtain walls were generally made of masonry; they had the solid look of bearing walls. Today, however, curtain walls are often made of lightweight materials such as glass, aluminum, or plastic, in various combinations. 由于现代结构重量是由钢或混凝土框架进行,墙壁不支持建设;他们已成为玻璃幕墙,它保持了天气和光线让;在早期的钢或混凝土框架结构,玻璃幕墙,一般由砖石,他们有坚实的承重墙看看;然而,今天,玻璃幕墙往往是由诸如玻璃,铝,塑料或轻质材料,在各种组合;Another advance in steel construction is the method of fastening together the beams. For many years the standard method was riveting. A rivet is abolt with a head that looks like a blunt screw without threads. It is heated, placed in holes through the pieces of steel, and a second head is formed at the other end by hammering it to hold it in place. Riveting has now largely been replaced by welding, thejoining together of pieces of steel by melting a steel material between them under high heat.钢结构建筑的另一个进步是梁紧固在一起的方法;多年来,标准方法是铆;铆钉是一个头,像一个没有线程看起来钝螺丝螺栓;它被加热时,通过放置在洞的钢件,第二头在另一端形成的锤击它举行到位;铆接现在很大程度上是由焊接取代,加入钢件在一起融化在高温下它们之间的钢铁材料;Presstressed concrete is an improved form of reinforcement. Steel rodsare bent into the shapes to give them the necessary degree of tensile strengths. They are then used to priestess concrete, usually by one of two different methods. The first is to leave channels in a concrete beam that correspond to the shapes of the steel rods. When the rods are run throughthe channels, they are then bonded to the concrete by filling the channels with grout, a thin mortar or binding agent. In the other and more common method, the priestesses steel rods are placed in the lower part of a formthat corresponds to the shape of the finished structure, and the concrete is poured around them. Priestess’s concrete uses less steel and less concrete. Because it is a highly desirable material.预应力钢筋混凝土是一种改进形式;棒钢弯曲成的形状,给他们一定程度的拉伸强度;然后他们用女祭司混凝土,由两种不同的方法之一,通常;首先是留在渠道混凝土梁对应于钢铁棒的形状;当棒是通过渠道来说,他们是那么粘在混凝土充填灌浆,薄砂浆或结合剂的渠道;在其他更常见的方法,女祭司钢棒放置在一个表格对应的成品下部结构形状,和他们周围的混凝土浇;女祭司的具体使用较少的钢铁和混凝土少;因为它是一个非常可取的材料;Presstressed concrete has made it possible to develop buildings with unusual shapes, like some of the modern, sports arenas, with large spaces unbroken by any obstructing supports. The uses for this relatively new structural method are constantly being developed. 预应力混凝土使人们有可能发展不寻常的形状的建筑物,如现代,体育场一些大空间的任何阻挠支持不间断;在使用这种相对较新的构造方法正在不断发展;。
土木工程英文文献及翻译

Civil engineeringCivil engineering is a professional engineering discipline that deals with the design, construction, and maintenance of the physical and naturally built environment, including works like bridges, roads, canals, dams, and buildings.[1][2][3] Civil engineering is the oldest engineering discipline after military engineering,[4] and it was defined to distinguish non-military engineering from military engineering.[5] It is traditionally broken into several sub-disciplines including environmental engineering, geotechnical engineering, structural engineering, transportation engineering, municipal or urban engineering, water resources engineering, materials engineering, coastal engineering,[4] surveying, and construction engineering.[6] Civil engineering takes place on all levels: in the public sector from municipal through to national governments, and in the private sector from individual homeowners through to international companies.History of the civil engineering professionSee also: History of structural engineeringEngineering has been an aspect of life since the beginnings of human existence. The earliest practices of Civil engineering may have commenced between 4000 and 2000 BC in Ancient Egypt and Mesopotamia (Ancient Iraq) when humans started to abandon a nomadic existence, thus causing a need for the construction of shelter. During this time, transportation became increasingly important leading to the development of the wheel and sailing.Until modern times there was no clear distinction between civil engineering and architecture, and the term engineer and architect were mainly geographical variations referring to the same person, often used interchangeably.[7]The construction of Pyramids in Egypt (circa 2700-2500 BC) might be considered the first instances of large structure constructions. Other ancient historic civil engineering constructions include the Parthenon by Iktinos in Ancient Greece (447-438 BC), theAppian Way by Roman engineers (c. 312 BC), the Great Wall of China by General Meng T'ien under orders from Ch'in Emperor Shih Huang Ti (c. 220 BC)[6] and the stupas constructed in ancient Sri Lanka like the Jetavanaramaya and the extensive irrigation works in Anuradhapura. The Romans developed civil structures throughout their empire, including especially aqueducts, insulae, harbours, bridges, dams and roads.In the 18th century, the term civil engineering was coined to incorporate all things civilian as opposed to military engineering.[5]The first self-proclaimed civil engineer was John Smeaton who constructed the Eddystone Lighthouse.[4][6]In 1771 Smeaton and some of his colleagues formed the Smeatonian Society of Civil Engineers, a group of leaders of the profession who met informally over dinner. Though there was evidence of some technical meetings, it was little more than a social society.In 1818 the Institution of Civil Engineers was founded in London, and in 1820 the eminent engineer Thomas Telford became its first president. The institution received a Royal Charter in 1828, formally recognising civil engineering as a profession. Its charter defined civil engineering as:the art of directing the great sources of power in nature for the use and convenience of man, as the means of production and of traffic in states, both for external and internal trade, as applied in the construction of roads, bridges, aqueducts, canals, river navigation and docks for internal intercourse and exchange, and in the construction of ports, harbours, moles, breakwaters and lighthouses, and in the art of navigation by artificial power for the purposes of commerce, and in the construction and application of machinery, and in the drainage of cities and towns.[8] The first private college to teach Civil Engineering in the United States was Norwich University founded in 1819 by Captain Alden Partridge.[9] The first degree in Civil Engineering in the United States was awarded by Rensselaer Polytechnic Institute in 1835.[10] The first such degree to be awarded to a woman was granted by Cornell University to Nora Stanton Blatchin 1905.History of civil engineeringCivil engineering is the application of physical and scientific principles, and its history is intricately linked to advances in understanding of physics and mathematics throughout history. Because civil engineering is a wide ranging profession, including several separate specialized sub-disciplines, its history is linked to knowledge of structures, materials science, geography, geology, soils, hydrology, environment, mechanics and other fields.Throughout ancient and medieval history most architectural design and construction was carried out by artisans, such as stone masons and carpenters, rising to the role of master builder. Knowledge was retained in guilds and seldom supplanted by advances. Structures, roads and infrastructure that existed were repetitive, and increases in scale were incremental.[12]One of the earliest examples of a scientific approach to physical and mathematical problems applicable to civil engineering is the work of Archimedes in the 3rd century BC, including Archimedes Principle, which underpins our understanding of buoyancy, and practical solutions such as Archimedes' screw. Brahmagupta, an Indian mathematician, used arithmetic in the 7th century AD, based on Hindu-Arabic numerals, for excavation (volume) computations.[13]Civil engineers typically possess an academic degree with a major in civil engineering. The length of study for such a degree is usually three to five years and the completed degree is usually designated as a Bachelor of Engineering, though some universities designate the degree as a Bachelor of Science. The degree generally includes units covering physics, mathematics, project management, design and specific topics in civil engineering. Initially such topics cover most, if not all, of thesub-disciplines of civil engineering. Students then choose to specialize in one or more sub-disciplines towards the end of the degree.[14]While anUndergraduate (BEng/BSc) Degree will normally provide successful students with industry accredited qualification, some universities offer postgraduate engineering awards (MEng/MSc) which allow students to further specialize in their particular area of interest within engineering.[15]In most countries, a Bachelor's degree in engineering represents the first step towards professional certification and the degree program itself is certified by a professional body. After completing a certified degree program the engineer must satisfy a range of requirements (including work experience and exam requirements) before being certified. Once certified, the engineer is designated the title of Professional Engineer (in the United States, Canada and South Africa), Chartered Engineer (in most Commonwealth countries), Chartered Professional Engineer (in Australia and New Zealand), or European Engineer (in much of the European Union). There are international engineering agreements between relevant professional bodies which are designed to allow engineers to practice across international borders.The advantages of certification vary depending upon location. For example, in the United States and Canada "only a licensed engineer may prepare, sign and seal, and submit engineering plans and drawings to a public authority for approval, or seal engineering work for public and private clients.".[16]This requirement is enforced by state and provincial legislation such as Quebec's Engineers Act.[17]In other countries, no such legislation exists. In Australia, state licensing of engineers is limited to the state of Queensland. Practically all certifying bodies maintain a code of ethics that they expect all members to abide by or risk expulsion.[18] In this way, these organizations play an important role in maintaining ethical standards for the profession. Even in jurisdictions where certification has little or no legal bearing on work, engineers are subject to contract law. In cases where an engineer's work fails he or she may be subject to the tort of negligence and, in extreme cases, thecharge of criminal negligence.[citation needed] An engineer's work must also comply with numerous other rules and regulations such as building codes and legislation pertaining to environmental law.CareersThere is no one typical career path for civil engineers. Most people who graduate with civil engineering degrees start with jobs that require a low level of responsibility, and as the new engineers prove their competence, they are trusted with tasks that have larger consequences and require a higher level of responsibility. However, within each branch of civil engineering career path options vary. In some fields and firms, entry-level engineers are put to work primarily monitoring construction in the field, serving as the "eyes and ears" of senior design engineers; while in other areas, entry-level engineers perform the more routine tasks of analysis or design and interpretation. Experienced engineers generally do more complex analysis or design work, or management of more complex design projects, or management of other engineers, or into specialized consulting, including forensic engineering.In general, civil engineering is concerned with the overall interface of human created fixed projects with the greater world. General civil engineers work closely with surveyors and specialized civil engineers to fit and serve fixed projects within their given site, community and terrain by designing grading, drainage, pavement, water supply, sewer service, electric and communications supply, and land divisions. General engineers spend much of their time visiting project sites, developing community consensus, and preparing construction plans. General civil engineering is also referred to as site engineering, a branch of civil engineering that primarily focuses on converting a tract of land from one usage to another. Civil engineers typically apply the principles of geotechnical engineering, structural engineering, environmental engineering, transportation engineering and construction engineering toresidential, commercial, industrial and public works projects of all sizes and levels of construction翻译:土木工程土木工程是一个专业的工程学科,包括设计,施工和维护与环境的改造,涉及了像桥梁,道路,河渠,堤坝和建筑物工程交易土木工程是最古老的军事工程后,工程学科,它被定义为区分军事工程非军事工程的学科它传统分解成若干子学科包括环境工程,岩土工程,结构工程,交通工程,市或城市工程,水资源工程,材料工程,海岸工程,勘测和施工工程等土木工程的范围涉及所有层次:从市政府到国家,从私人部门到国际公司。
土木工程专业外文文献及翻译

土木工程专业外文文献及翻译外文文献及翻译学号:学校代码:(二〇一二年六月题目: About Buiding on the Structure Design 学生姓名:学院:土木工程学院系别:建筑工程系专业:土木工程(建筑工程方向) 班级:土木08-4班指导教师:英文原文:Building construction concrete crack ofprevention and processingAbstractThe crack problem of concrete is a widespread e某istencebut again difficult in solve of engineering actual problem, this te某t carried on a study analysis to a little bit familiarcrack problem in the concrete engineering, and aim at concretethe circumstance put forward some prevention, processing measure. Keyword:Concrete crack prevention processingForewordConcrete's ising 1 kind is anticipate by the freestone bone, cement, water and other mi某ture but formation of the inaddition material of quality brittleness not andall the concrete construction transform with oneself,control etc. a series problem, harden model of in the concrete e某istence numerous tiny hole, spirit cave and tiny crack, is e某actly because these beginning start blemish of e某istencejust make the concrete present one some not and all the characteristic of tiny crack is a kind of harmless crack and accept concrete heavy, defend Shen and a little bit other use function not a creation to after the concrete be subjected to lotus carry, difference in temperature etc. function, tiny crack would continuously of e某pand with connect, end formation we can see without the aid of instruments of macro view the crack be also the crack that the concrete often say in the engineering.Concrete building and Gou piece usually all take sewer to make of, because of crack of e某istence and development usually make inner part of reinforcing bar etc. material creation decay, lower reinforced concrete material of loading ability, durable and anti- Shen ability, influence building of e某ternal appearance, service life, severity will threat arrive people's life and property lot of all of crash of engineerings is because of the unsteady development of the crack with the result age science research with a great deal of of the concrete engineering practice certificate, in the concrete engineering crack problem is ineluctable, also acceptable in certainly ofthe scope just need to adopt valid of measure will it endanger degree control at certain of scope reinforced concrete norm is also e某plicit provision:Some structure at place ofdissimilarity under the condition allow e某istence certain the crack of at under construction should as far as possible adopt a valid measure control crack creation, make the structure don'tappear crack possibly or as far as possible decrease crack of amount and width, particularly want to as far as possible avoid harmful crack of emergence, insure engineering quality thus.Concrete crack creation of the reason be a lot of and have already transformed to cause of crack:Such as temperature variety, constringency, inflation, the asymmetry sink to sink etc. reason cause of crack;Have outside carry the crack that the function cause;Protected environment not appropriate the crack etc. caused with chemical differentiation to treat in the actual engineering, work°out a problem according to the actual circumstance.In the concrete engineering the familiar crack and the prevention Suo crack and preventionSu constringency crack and preventionto sink crack and preventionThe creation which sink to sink crack is because of the structure foundation soil quality not and evenly, loose soft or return to fill soil dishonest or soak in water but result in the asymmetry sink to decline with the result that;Perhaps because of template just degree shortage, the template propped up to once be apart from big or prop up bottom loose move etc. to cause, especially at winter, the template prop up at jelly soil up, jelly the soil turn jelly empress creation asymmetry to sink to decline and cause concrete structure creation kind crack manyis deep enter or pierce through se某 crack, it alignment have something to do with sinking to sink a circumstance, general follow with ground perpendicular or present 30 °s-45 ° Cape direction development, bigger sink to sink crack, usually have certain of wrong, crack width usually with sink to decline quantity direct proportion width under the influence of temperature variety foundation after transform stability sink to sink crack also basic tend in stability.crack and prevention。
土木工程专业英语带译文

Chapter 6
If a material with high strength in tension, such as steel, is placed in concrete, then the composite material, reinforced concrete, resists not only compression but also bending and other direct tensile actions. A reinforced concrete section where the concrete resists the compression and steel resists the tension can be made into almost any shape and size for the construction industry.
6. —We shall finish the civil work by the end of the year. 在年底前我们将完成土建工作。 —Cement steel and timber are the most important construction materials used in civil engineering. 水泥、钢材和木材是土建工程中最重要的建筑材料。 7. These are the anchor bolts (rivets, unfinished bolts, high-strength structural bolts) for the structure. 这是用于结构的锚定螺栓(铆钉、粗制螺栓、高强度结构用螺栓)。
Chapter 6
Chapter 6 Reinforced Concrete
土木工程--外文文献翻译

土木工程--外文文献翻译-CAL-FENGHAI.-(YICAI)-Company One1学院:专业:土木工程姓名:学号:外文出处: Structural Systems to resist (用外文写)Lateral loads附件: 1.外文资料翻译译文;2.外文原文。
附件1:外文资料翻译译文抗侧向荷载的结构体系常用的结构体系若已测出荷载量达数千万磅重,那么在高层建筑设计中就没有多少可以进行极其复杂的构思余地了。
确实,较好的高层建筑普遍具有构思简单、表现明晰的特点。
这并不是说没有进行宏观构思的余地。
实际上,正是因为有了这种宏观的构思,新奇的高层建筑体系才得以发展,可能更重要的是:几年以前才出现的一些新概念在今天的技术中已经变得平常了。
如果忽略一些与建筑材料密切相关的概念不谈,高层建筑里最为常用的结构体系便可分为如下几类:1.抗弯矩框架。
2.支撑框架,包括偏心支撑框架。
3.剪力墙,包括钢板剪力墙。
4.筒中框架。
5.筒中筒结构。
6.核心交互结构。
7. 框格体系或束筒体系。
特别是由于最近趋向于更复杂的建筑形式,同时也需要增加刚度以抵抗几力和地震力,大多数高层建筑都具有由框架、支撑构架、剪力墙和相关体系相结合而构成的体系。
而且,就较高的建筑物而言,大多数都是由交互式构件组成三维陈列。
将这些构件结合起来的方法正是高层建筑设计方法的本质。
其结合方式需要在考虑环境、功能和费用后再发展,以便提供促使建筑发展达到新高度的有效结构。
这并不是说富于想象力的结构设计就能够创造出伟大建筑。
正相反,有许多例优美的建筑仅得到结构工程师适当的支持就被创造出来了,然而,如果没有天赋甚厚的建筑师的创造力的指导,那么,得以发展的就只能是好的结构,并非是伟大的建筑。
无论如何,要想创造出高层建筑真正非凡的设计,两者都需要最好的。
虽然在文献中通常可以见到有关这七种体系的全面性讨论,但是在这里还值得进一步讨论。
设计方法的本质贯穿于整个讨论。
土木工程专业英语课文原文及对照翻译

土木工程专业英语课文原文及对照翻译土木工程师建造道路、桥梁、隧道、水坝、港口、发电厂、水和污水系统、医院、学校、大众交通和其他对现代社会和大量人口集中地区至关重要的公共设施。
他们还建造私人拥有的设施,如机场、铁路、管道、摩天大楼和其他为工业、商业或住宅使用而设计的大型结构。
此外,土木工程师规划、设计和建造完整的城市和城镇,最近还在规划和设计太空平台,以容纳自给自足的社区。
___ passes the planning。
design。
n。
and management of the built ___ scientific principles。
from ___ are essential to modern society。
such as roads。
bridges。
___。
dams。
and hospitals.___ public facilities。
civil engineers also design and build privately-owned structures。
including airports。
railroads。
pipelines。
skyscrapers。
and other ___。
and ___.Overall。
___ civil engineers。
our modern infrastructure and public facilities would not exist.___。
n。
and maintenance of public and private infrastructure。
This includes roads。
bridges。
pipelines。
dams。
ports。
power plants。
water supply and sewage systems。
hospitals。
schools。
___。
and other structures that are essential to modern ___ as airports。
土木工程英语文献原文及中文翻译

Civil engineering introduction papers[英语原文]Abstract: the civil engineering is a huge discipline, but the main one is building, building whether in China or abroad, has a long history, long-term development process. The world is changing every day, but the building also along with the progress of science and development. Mechanics findings, material of update, ever more scientific technology into the building. But before a room with a tile to cover the top of the house, now for comfort, different ideas, different scientific, promoted the development of civil engineering, making it more perfect.[key words] : civil engineering; Architecture; Mechanics, Materials.Civil engineering is build various projects collectively. It was meant to be and "military project" corresponding. In English the history of Civil Engineering, mechanical Engineering, electrical Engineering, chemical Engineering belong to to Engineering, because they all have MinYongXing. Later, as the project development of science and technology, mechanical, electrical, chemical has gradually formed independent scientific, to Engineering became Civil Engineering of specialized nouns. So far, in English, to Engineering include water conservancy project, port Engineering, While in our country, water conservancy projects and port projects also become very close and civil engineering relatively independent branch. Civil engineering construction of object, both refers to that built on the ground, underground water engineering facilities, also refers to applied materials equipment and conduct of the investigation, design and construction, maintenance, repair and other professional technology.Civil engineering is a kind of with people's food, clothing, shelter and transportation has close relation of the project. Among them with "live" relationship is directly. Because, to solve the "live" problem must build various types of buildings. To solve the "line, food and clothes" problem both direct side, but also a indirect side. "Line", must build railways, roads, Bridges, "Feed", must be well drilling water, water conservancy, farm irrigation, drainage water supply for the city, that is direct relation. Indirectly relationship is no matter what you do, manufacturing cars, ships, or spinning and weaving, clothing, or even production steel, launch satellites, conducting scientific research activities are inseparable from build various buildings, structures and build all kinds of project facilities.Civil engineering with the progress of human society and development, yet has evolved into large-scale comprehensive discipline, it has out many branch, such as: architectural engineering, the railway engineering, road engineering, bridge engineering, special engineering structure, waterand wastewater engineering, port engineering, hydraulic engineering, environment engineering disciplines. [1]Civil engineering as an important basic disciplines, and has its important attributes of: integrated, sociality, practicality, unity. Civil engineering for the development of national economy and the improvement of people's life provides an important material and technical basis, for many industrial invigoration played a role in promoting, engineering construction is the formation of a fixed asset basic production process, therefore, construction and real estate become in many countries and regions, economic powerhouses.Construction project is housing planning, survey, design, construction of the floorboard. Purpose is for human life and production provide places.Houses will be like a man, it's like a man's life planning environment is responsible by the planners, Its layout and artistic processing, corresponding to the body shape looks and temperament, is responsible by the architect, Its structure is like a person's bones and life expectancy, the structural engineer is responsible, Its water, heating ventilation and electrical facilities such as the human organ and the nerve, is by the equipment engineer is responsible for. Also like nature intact shaped like people, in the city I district planning based on build houses, and is the construction unit, reconnaissance unit, design unit of various design engineers and construction units comprehensive coordination and cooperation process.After all, but is structural stress body reaction force and the internal stress and how external force balance. Building to tackle, also must solve the problem is mechanical problems. We have to solve the problem of discipline called architectural mechanics. Architectural mechanics have can be divided into: statics, material mechanics and structural mechanics three mechanical system. Architectural mechanics is discussion and research building structure and component in load and other factors affecting the working condition of, also is the building of intensity, stiffness and stability. In load, bear load and load of structure and component can cause the surrounding objects in their function, and the object itself by the load effect and deformation, and there is the possibility of damage, but the structure itself has certain resistance to deformation and destruction of competence, and the bearing capacity of the structure size is and component of materials, cross section, and the structural properties of geometry size, working conditions and structure circumstance relevant. While these relationships can be improved by mechanics formula solved through calculation.Building materials in building and has a pivotal role. Building material is with human society productivity and science and technologyimproves gradually developed. In ancient times, the human lives, the line USES is the rocks andTrees. The 4th century BC, 12 ~ has created a tile and brick, humans are only useful synthetic materials made of housing. The 17th century had cast iron and ShouTie later, until the eighteenth century had Portland cement, just make later reinforced concrete engineering get vigorous development. Now all sorts of high-strength structural materials, new decoration materials and waterproof material development, criterion and 20th century since mid organic polymer materials in civil engineering are closely related to the widely application. In all materials, the most main and most popular is steel, concrete, lumber, masonry. In recent years, by using two kinds of material advantage, will make them together, the combination of structure was developed. Now, architecture, engineering quality fit and unfit quality usually adopted materials quality, performance and using reasonable or not have direct connection, in meet the same technical indicators and quality requirements, under the precondition of choice of different material is different, use method of engineering cost has direct impact.In construction process, building construction is and architectural mechanics, building materials also important links. Construction is to the mind of the designer, intention and idea into realistic process, from the ancient hole JuChao place to now skyscrapers, from rural to urban country road elevated road all need through "construction" means. A construction project, including many jobs such as dredging engineering, deep foundation pit bracing engineering, foundation engineering, reinforced concrete structure engineering, structural lifting project, waterproofing, decorate projects, each type of project has its own rules, all need according to different construction object and construction environment conditions using relevant construction technology, in work-site.whenever while, need and the relevant hydropower and other equipment composition of a whole, each project between reasonable organizing and coordination, better play investment benefit. Civil engineering construction in the benefit, while also issued by the state in strict accordance with the relevant construction technology standard, thus further enhance China's construction level to ensure construction quality, reduce the cost for the project.Any building built on the surface of the earth all strata, building weight eventually to stratum, have to bear. Formation Support building the rocks were referred to as foundation, and the buildings on the ground and under the upper structure of self-respect and liable to load transfer to the foundation of components or component called foundation. Foundation, and the foundation and the superstructure is a building of three inseparable part. According to the function is different, but in load, under the action of them are related to each other, is theinteraction of the whole. Foundation can be divided into natural foundation and artificial foundation, basic according to the buried depth is divided into deep foundation and shallow foundation. , foundation and foundation is the guarantee of the quality of the buildings and normal use close button, where buildings foundation in building under loads of both must maintain overall stability and if the settlement of foundation produce in building scope permitted inside, and foundation itself should have sufficient strength, stiffness and durability, also consider repair methods and the necessary foundation soil retaining retaining water and relevant measures. [3]As people living standard rise ceaselessly, the people to their place of building space has become not only from the number, and put forward higher requirement from quality are put car higher demands that the environment is beautiful, have certain comfort. This needs to decorate a building to be necessary. If architecture major engineering constitutes the skeleton of the building, then after adornment building has become the flesh-and-blood organism, final with rich, perfect appearance in people's in front, the best architecture should fully embody all sorts of adornment material related properties, with existing construction technology, the most effective gimmick, to achieve conception must express effect. Building outfit fix to consider the architectural space use requirement, protect the subject institutions from damage, give a person with beautifulenjoying, satisfy the requirements of fire evacuation, decorative materials and scheme of rationality, construction technology and economic feasibility, etc. Housing construction development and at the same time, like housing construction as affecting people life of roads, Bridges, tunnels has made great progress.In general civil engineering is one of the oldest subjects, it has made great achievements, the future of the civil engineering will occupy in people's life more important position. The environment worsening population increase, people to fight for survival, to strive for a more comfortable living environment, and will pay more attention to civil engineering. In the near future, some major projects extimated to build, insert roller skyscrapers, across the oceanBridges, more convenient traffic would not dream. The development of science and technology, and the earth is deteriorating environment will be prompted civil engineering to aerospace and Marine development, provide mankind broader space of living. In recent years, engineering materials mainly is reinforced concrete, lumber and brick materials, in the future, the traditional materials will be improved, more suitable for some new building materials market, especially the chemistry materials will promote the construction of towards a higher point. Meanwhile, design method of precision, design work of automation, information and intelligent technology of introducing, will be people have a morecomfortable living environment. The word, and the development of the theory and new materials, the emergence of the application of computer, high-tech introduction to wait to will make civil engineering have a new leap.This is a door needs calm and a great deal of patience and attentive professional. Because hundreds of thousands, even hundreds of thousands of lines to building each place structure clearly reflected. Without a gentle state of mind, do what thing just floating on the surface, to any a building structure, to be engaged in business and could not have had a clear, accurate and profound understanding of, the nature is no good. In this business, probably not burn the midnight oil of courage, not to reach the goal of spirit not to give up, will only be companies eliminated.This is a responsible and caring industry. Should have a single responsible heart - I one's life in my hand, thousands of life in my hand. Since the civil, should choose dependably shoulder the responsibility.Finally, this is a constant pursuit of perfect industry. Pyramid, spectacular now: The Great Wall, the majestic... But if no generations of the pursuit of today, we may also use the sort of the oldest way to build this same architecture. Design a building structure is numerous, but this is all experienced centuries of clarification, through continuous accumulation, keep improving, innovation obtained. And such pursuit, not confined in the past. Just think, if the design of a building can be like calculation one plus one equals two as simple and easy to grasp, that was not for what? Therefore, a civil engineer is in constant of in formation. One of the most simple structure, the least cost, the biggest function. Choose civil, choosing a steadfast diligence, innovation, pursuit of perfect path.Reference:[1] LuoFuWu editor. Civil engineering (professional). Introduction to wuhan. Wuhan university of technology press. 2007[2] WangFuChuan, palace rice expensive editor. Construction engineering materials. Beijing. Science and technology literature press. 2002[3] jiang see whales, zhiming editor. Civil engineering introduction of higher education press. Beijing.. 1992土木工程概论 [译文]摘要:土木工程是个庞大的学科,但最主要的是建筑,建筑无论是在中国还是在国外,都有着悠久的历史,长期的发展历程。
土木工程外文翻译5

附录(一)外文原文4.2.1.1 C ement Test by Sieve No. 170The fineness of cement affects the quality of the concrete industry in general. A big cement particle cannot completely react with water as water cannot reach a remaining core in the cement particle. The water propagates through the cement particles and they start to dehydrate, which causes an increase in temperature, which is the main reason for the forming of hair cracks and preventing stabilization of cement volume. As a result, an increase in the cement particle size reduces the strength of the same cement content and increasing the fineness of the cement will improve the workability,cohesion, and durability with time and decrease the water moving upward to the concrete surface.Figure 4.1, from Neville’s book (1983), presents the relation between concrete strength and the concrete fineness at different ages. To perform this test, take a sample of 50 g of cement and shake it in a closed glass bottle for two minutes and then revolve the sample gently using dry bar. Put the sample in a closed bottle and leaveit for two minutes. Put the sample in 170 sieve (90 microns) and move it, shakingthe sieve horizontally and rotationally, then confirm finishing the sieve test when the rate of passing cement particles is not more than 0.5 g/min during the sieve process. Remove the fines carefully from the bottom of the sieve using a smooth brush. Then, collect and weigh the remaining particles on the sieve (W1).Repeat the same test with another sample. Then the residual weight for the second test is obtained (W2). Calculate the values of the remaining samples throughR1 = (W1/50) ×100R2 = (W2/50) ×100The ratio (R) is calculated by taking the average of R1 and R2 to the nearest 0.1% and, in the case of deviating results of the two samples, more than 1%. Do the test a third time and take the average of the three results.You can accept or refuse the cement based on the following condition:For Portland cement t •he R must not exceed than 10%.•For rapid hardening Portland cement the R must not exceed 5%.4.2.1.2 Initial and Final Setting Times of CementPaste Using Vicat ApparatusThe objective of this test is to define the time for initial and final setting of the paste of water and cement with standard consistency by using a Vicat apparatus and determinewhether the cement is expired or can be used.The initial setting is the required time to set and after that concrete cannot be poured or formed; the final setting time is the time required for the concrete to be hardened.Vicat apparatus (Figure 4.2) consists of a carrier with needle acting under a prescribed weight. The parts move vertically without friction and are not subject to erosionor corrosion. The paste mold is made from a metal or hard rubber or plastic likea cut cone with depth of 40 ±2 mm and the internal diameter of the upper face 70 ±5 mm and lower face 80 ±5 mm and provides a template of glass or similar materialsin the softer surface. Its dimensions are greater than the dimensions of the mold.The needle is used to determine the initial setting time in a steel cylinder with effective length 50 ±1 mm and diameter 1.13 ±0.5 mm. The needle measuring timeis in the form of a cylinder with length of 30 ±1 mm and diameter 1.13 ±0.5 mm andheld by a 5 mm diameter ring at the free end to achieve distance between the end of the needle and the ring of 0.5 mm.The test starts by taking a sample weighing about 400 g and placing it on an impermeable surface and then adding 100 ml of water and recording zero measurementfrom the time of adding water to the cement and then mixing for 240 + 5 secondson the impermeable surface.To determine the initial setting time and calibrate the device until the needle reaches the base of the mold, then adjust the measuring device to zero and return needle to its original place.Fill the mold with cement paste with standard consistency and troll the surface,then put the mold for a short time in a place that has the the temperature and humidity required for the test.Specific Surface (Wagner)-m2/kg365 days90 days28 days7 days20150 200 250 300304050Compressive Strength, MpaTransfer the mold to the apparatus under the needle, and then make the needleslowly approach the surface until it touches the paste’s surface, stop it in place fora second or two seconds to avoid impact of primary speed, then allow the moving parts to implement the needle vertically in the paste.Grading depends on when the needle stops penetrating or after 30 seconds, whichever is earlier, and indicates the distance between the mold base and the end of the needle, as well as the time start from the zero level measurement.Repeat the process of immersing the needle in the same paste in different locations with the distance between the immersing point and the edge of the mold orbetween two immersing points not less 10 mm after about 10 minutes, and clean the needle immediately after each test.Record time is measured from zero up to 5 ±1 mm from the base of the mold as the initial setting time to the nearest 5 minutes. Ensuring the accuracy of measurement of time between tests reduces embedment and the fluctuation of successivetests. The needle is used to identify the final time of setting; follow the same stepsas in determining time of initial setting and increase the period between embedment tests to 30 minutes.Record the time from zero measurement until embedment of the needle to a distance of 0.5 mm, which will be the final setting time. Control the impact of theneedle on the surface of the sample so the final setting time presents the effect ofthe needle. To enhance the test’s accuracy reduce the time between embedment tests and examine the fluctuation of these successive tests. Record the final setting timeto the nearest 5 mm.According to the Egyptian specifications the initial setting time must not be lessthan 45 minutes for all types of cement except the low heat cement, for which the initial setting time must not be less 60 minutes. The final setting time must be shorter than 10 hours for all types of cement.4.2.1.3 D ensity of CementThe purpose of this test is to determine the density of cement by identifying the weight and unit volume of the material by using the Le Chatelier density bottle. The determination of the cement density is essential for concrete mix design and to control its quality. This test follows specifications of the American Society for Testingand Materials, ASTM C188-84.The Le Chatelier device is a standard round bottle. Its shape and dimensions are shown in Figure 4.3. This bottle must have all the required dimensions, lengths, and uniform degradation and accuracy.The glass that is used in the Le Chatelier bottle must be of high quality and freefrom any defects. It should not interact with chemicals and have high resistance to heat and appropriate thickness to have a high resistance to crushing. Measurements start at the bottle’s neck and go from zero to 1 mL and from 18 to 24 mL with accuracyto 0.1 mL. Each bottle must have a number to distinguish it from any other.Write on the bottle the standard temperature and the capacity in millimeters over the highest point of grading.Processed sample cement weighing about 64 g to the nearest 0.05 g must be tested.Fill the bottle with kerosene free from water and oil whose density is at least 62 API. Up to point gradations between zero and 1 mL, dry the inner surface of the bottle at the highest level of kerosene if necessary, and use rubber on the surface of the table used for the test when filling the bottle.The bottle, which is filled with kerosene, is placed in a water bath and the firstreading to kerosene level is recorded. To record the first reading correctly install the bottle in the water bath vertically. Put a cement sample weighing 64 g with accuracy to 0.05 g inside the bottle with small batches at the same temperature of kerosene, taking into account when putting the cement inside the bottle to avoid cement droppingout or its adhesion on the internal surfaces of the bottle at the highest level. The bottle can be placed on the vibrating machine when putting the cement inside the bottle to expedite the process and prevent adhesion of granulated cement with the internal surfaces of the bottle.After laying the cement inside the bottle, put a cap on the bottle mouth and then spin diagonally on the surface so as to expel the air between the granules of cement, and continue moving the bottle until the emergence of air bubbles stops from the kerosene surface inside the bottle.Put the bottle in the water bath and then take the final reading, and record the reading at the lower surface of kerosene so as to avoid the impact of surface tension. For the first and final readings, make sure that the bottle is placed in a water bath with constant temperature for a period not to exceed the difference in temperature between the first and final readings of about 0.2°C.The difference between the first and final reading is the volume of the moving liquid by the cement sample.The volume of the moving liquid = final reading –first reading4.2.1.4 D efine Cement Fineness by Using Blaine ApparatusThis test is used to determine the surface area by comparing the test sample with the specific reference. The greater surface area increases the speed of concrete hardening and obtains early strength. This test determines the acceptance of the cement. There are many tests to define cement fineness and one is a Blaine apparatus as stated in many codes such as the Egyptian code.This test depends on calculating the surface area by comparing the sample test and the reference sample using a Blaine apparatus to determine the time required to pass a definite quantity of air inside a cement layer with defined dimensions and porosity.A Blaine apparatus is shown in Figure 4.4. The first step in testing is to determine the volume of the cement layer using mercury in the ring device of the Blaine apparatus.Cement is then added and by knowing the weight of the cement before andafter adding it as well as the mercury density, the volume of the cement layer can be calculated.V = W1 –W2/DmwhereV is the volume of cement layer, cm3.Fi gure 4.4 Blaine apparatus.Concrete Materials and Tests 111W1 is the weight of mercury in grams that fills the device to nearest (0.0 g).W2 is the weight of mercury in grams that fills the device to nearest (0.0 g).Dm is the density of the mercury (g/cm3). From tables, define the mercury densityat the average temperature of the test by using the manometer in the Blaine apparatus.From the previous equation:Sr is the reference cement surface area, (cm2/g).Dr is the reference cement density (g/cm3).Pr is the porosity of the cement layer.Ir is the air visciosity in the average temperature for reference cement test.Tr is the average time required for the manometer liquid to settle in two marksto nearest 0.2 sec.K is the Blaine apparatus constant factor defined by the previous equation by knowing the time needed to pass the air in the sample.To retest the sample, we calculate its surface area by using the following equation:Sc = Sr(Dr/Dc) *(Tc/Tr)^0.5According to the Egyptian code, the acceptance and refusal of cement is based on limites shown in Table 4.2.Table 4.2Cement Fineness Acceptance andRefusal LimitsCement TypesCement Fineness Not LessThan cm2/gmOrdinary Portland 2750Rapid hardening Portland 3500Sulfate resistant Portland 2800Low heat Portland 2800White Portland 2700Mixing sand Portland 30004100 fineness 4100Slag Portland 25004.2.1.5 C ompressive Strength of Cement MortarsThe cement mortar compressive strength test is performed using standard cubesof cement mortar mixed manually and compacted mechanically using a standard vibrating machine. This test is considered a refusal or acceptance determination. Compressive strength is one of the most important properties of concrete. The concrete gains its compressive strength from cement paste as a result of the interactionbetween the cement and water added to the mix. So it is critical to make sure that the cement used is the appropriate compressive strength. This test should be done to all types of cement.Needed for the test are stainless steel sieves with standard square holes opened 850 or 650 microns. Stainless steel does not react with cement and weighs 210 g. The vibrating machine has a weight of about 29 kg and the speed of vibration is about 12,000 vertical vibrations + 400 RPM and the moment of vibrating column is0.016 N.m.The mold of the test is a cube 70.7 ±1 mm, the surface area for each surface is500 mm2, the acceptable tolerance in leveling is about 0.03 mm, and the tolerance between paralleling for each face is about 0.06 mm.The mold is manufactured from materials that will not react with the cement mortar, and the base of the mold is made from steel that can prevent leaks of the mortaror water from the mold. The base is matched with the vibrating machine.The sand should contain a percentage of silica not less than 90% by weight andmust be washed and dried very well. Moreover, the humidity of the sand must not be more than 0.1% by weight for it to pass through a sieve with openings of 850 microns, and for it to pass through the standard sieve size of 600 microns it should not have more than 10% humidity by weight (Tables 4.3 and 4.4).After performing the tests, the standard cubes will be crushed within one day,which is about 24 ±0.5 hours, and three days in the limits of 72 ±1 hour, and afterseven days within 168 ±1 hour, and after 28 days within 672 ±1 hour.Table 4.5 illustrates the limits of acceptance and rejection according to the cement mortar compressive strength. Note from the table that there is more than one typeof high-alumina cement as the types vary according to the percentage of oxide alumina.The compressive strength after 28 days will not be considered accepted orrejected unless clearly stated in the contract between the supplier and the client(二)外文原文翻译4.2.1.1水泥试验筛170号水泥的优质一般影响混凝土行业的质量。
土木工程外文翻译

英文翻译1外文原文出处:School of Civil Engineering, Civil Engineering Materials Unit (CEMU), University of Leeds, Leeds LS2 9JT, UKReceived 10 February 2000;原文1Compatibility of repair mortars with concrete in a hot-dryenvironmentAbstractStrengthening, maintenance and repair of concrete structures are becoming more recognised in the field of civil engineering. There is a wide range of repair mortars with varying properties, available in the market and promoted by the suppliers, which makes the selection of the most suitable one often difficult. A research programme was conducted at Leeds University to investigate the properties of cementitious, polymer and polymer modified (PMC) repair mortars. Following an earlier publication on the intrinsic properties of the materials, this paper presents results on the compatibility of these materials with concrete. The dimensional stability is used in this study to investigate the compatibility of the repair mortars and the parent concrete. Composite cylindrical specimens (half repair mortar/half concrete)were prepared and used for the measurements of modulus of elasticity and shrinkage. The results of the different combined systems were obtained and compared to those calculated using a composite model. The variations between the measured and calculated values were less than 10%. The paper attempts to quantify the effect of indirect differential shrinkage on the permeability and diffusion characteristics of the different combined systems.Author Keywords:Compatibility; Concrete; Dimensional stability; Modulus of elasticity; Oxygen diffusion; Oxygen permeability; Repair mortars; Shrinkage1. IntroductionDeterioration of concrete structures is a major problem in civil engineering, which is mainly associated with contamination, cracks and spalling of the cover concrete. In many instances, the serviceability of the deteriorated structure becomes an important issue and therefore the most cost-effective solution is often to use patch repair, which involves the removal of the deteriorated parts and reinstatement with a fresh repair mortar . The effectiveness of this approach is influenced by the intrinsic properties of the selected repair material (to eliminate the cause of initial deterioration), the chloride contamination level of the concrete adjacent to the repaired zone [1 and 2] and the compatibility of the combined system (concrete/repair).Compatibility in a repair system is the combination of properties between the repair material and the existing concrete substrate which ensures that the combined system withstands the applied stresses and maintains its structural integrity and protective properties in a certain exposure environment over a designated service life [3and 4]. Dimensional stability, chemical, electrochemical, and transport properties of the repair material and the parent concrete are the main aspects of compatibility.The dimensional stability is probably the most important factor which controls the volume changes due to shrinkage, thermal expansion, and the effects of creep and modulus of elasticity [5, 6and 7]. The chemical and electrochemical properties include attack due to alkali silica reaction, sulphate content, pH, electrical resistivity, chloride and carbonation induced corrosion, whereas the permeability and diffusion characteristics of both materials and at the interface between them are the main consideration for a durable combined system.Previous studies [5 and 6] compared properties of various repair mortars and then used finite elements analysis to study the performance of axially loaded reinforced concrete.In this study the compatibility of five repair mortars and concrete,in terms of modulus of elasticity and shrinkage, was investigated . The paper reviews a simple model describing the modulus and shrinkage behaviour of composite materials and presents an experimental programme on the application of the model. It emphasises the indirect effect of differential shrinkage on the transport properties of the different combined systems.2. Model theoryLet the combined system of parent concrete and repair mortar be subjected to an external stress (σ0), have a modulus of elasticity –E0, Poisson's ratio –μ0and shrinkage –S0. The corresponding properties of the two phases are shown in (parent concrete:symbol ―c‖ and repair mortar: ―m‖).3. Experimental work3.1. Parent concreteThe control concrete mix had the composition of OPC:sand:gravel in the weight ratio of 1:2.33:3.5, with a cement content of 325 kg/m3. The sand grading conformed to zone M of BS 882 [9], and the gravel had a maximum size of 10 mm. The w/c used was 0.55, which resulted in a slump value of 55 mm.Cylindrical specimens (150 mm diameter and 300 mm height, and 75 mm diameter and 265 mm height) were cast for the dimensional stability study. Additional cubes (100 mm) and slabs (400×250×40 mm3) were also cast for studying the properties of the parent concrete.The specimens were demoulded after 24 h and cured in a fog room maintained at 20°C and 99% relative humidity (RH). The properties of the control concrete substrate, measured at the age of 28 days, are given in.The cylindrical concrete specimens were kept in the fog room for 3 months. This long curing period was chosen to provide a relatively old concrete substrate for the repair mortars. The cylinders were then split along their longitudinal axis into two halves following the procedure of BS 1881: Part 117 [10] for tensile splitting strength. The loose particles were removed and the fractured surfaces were cleaned using a wire brush. The split cylinders were transferred to the hot dry environmental chamber controlled at 35°C, 45% RH and 3 m/s wind velocity, and kept there for 7 days beforecasting the repair mortars.3.2. Repair mortarsFive repair materials were selected in this investigation. These include: conventional cementitious, epoxy resin (EP) and polymer modified mortars (PMC). Table 1gives details of the repair materials. The repair mortars used in the study are the same investigated in [11], where their intrinsic properties are reported.3.3. Combined specimensThe half cylinder specimens were sprayed with water and placed again into their original moulds. The other halves of the moulds were cast with the different repair mortars to produce combined specimens. The specimens were compacted and kept covered overnight with wet hessian and polyethylene sheets. After 24 h, the combined specimens were demoulded and cured for 27 days in the same hot dry environment (35°C, 45% RH and 3 m/s wind velocity).The 75-mm diameter cylinders were used for the measurements of shrinkage strains between 3 and 28 days. Demec points were attached to the combined cylinders, on both sides (concrete/repair material), at a gauge length of 200 mm. After 28 days, cores (50-mm diameter) were drilled to have one half of the repair mortar and the other of the parent concrete.These cores were used for studying the effect of differential shrinkage on the transport properties of the combined systems. shows details of the shrinkage cylinder with locations of the Demec points and the drilled cores.The (150-mm) cylinders were used for the measurements of compressive modulus of elasticity and strength at 28 days. Flat loading surfaces were produced by grinding the opposite faces of each cylinder. Strain gauges (20-mm length) were fixed on each side of the repair mortar and the parent concrete as shown in Fig. 4. The specimens were tested using the Tonipact-3000 (cube crushing machine), with a loading rate of 0.2 N/mm2/s. The top loading plate of the machine is initially free to level with the test specimens (up to 5 kN load), then locked automatically to minimise the effect of load eccentricity. Load-strain readings were recorded automatically using a computer data acquisition system.Duplicate specimens were used for each test and the average values were used in presenting the results. The variation of results was less than 10% for the engineering and shrinkage properties, and less than 25% for the transport properties. The testing procedures used for measuring shrinkage, modulus of elasticity and transport properties were similar to those used for testing the individual repair materials in [11].4. Presentation of results4.1. Modulus of elasticityThe modulus of elasticity is the property which controls the load distribution of a combined system composed of two materials. The elastic stress–strain behaviour (up to 1/3 of the failure load) of the individual repair mortars, concrete,and the average values of the combined systems (labelled as Comb) are presented in Figs. 5(a)–(e). The individual values are given in Table 3together with the measured average of the combined systems. Table 3gives also the moduli for the different combined systems (E0), as calculated from Eq. (8) using the individual values of E c and E m.Fig. 5. Stress–strain relationships for the different combined systems: (a) OPC/ concrete system; (b) FA/concrete system, the comb curve falls behind the conc curve; (c) SF/concrete system; (d) PMC/concrete system; (e) EP/concrete system.The results show that, except for the PMC and EP, the modulus values of the cementitious repair mortars are quite similar to that of the parent concrete. Consequently, when combined together, the modulus of OPC, FA and SF combined systems did not change much, indicating only a slight effect on the load distribution of the combined systems and hence modulus compatibility. In contrast to the cementitious mortars, the PMC and the EP mortars had different modulus values to that of the concrete.As a result of the combined action, the PMC repair mortar increased the modulus of concrete,whereas the EC mortar caused a reduction in the concrete modulus.When the values of modulus are compared, the combined (measured) modulus agreeswith the average modulus of the individual materials (E0) to within 10%. This is in compliance with the theory of combined modelling when there is no discontinuity of strain at the interface, for example, cracking and a transitional zone effect. It also suggests that the effect of Poisson's ratio as considered in the derivation of Eq. (6a) is not significant for the materials used.4.2. Compressive strengthFig. 6 shows the stress–strain relationships for the different combined cylinders up to failure, whereas the numerical values of the 28 days compressive strength for the individual repair mortars and the combined cylinders are given in Table 4. Although the PMC showed a relatively higher modulus than that of the concrete, its stress–strain behaviour when combined with the parent concrete was found to be quite similar to those of the cementitious mortars. In fact the compressive strength value for the PMC/concrete was slightly higher than that of the parent concrete. The EP/concrete system showed a different behaviour and exhibited a strength value of 38.4 MPa. This value is relatively low when compared with the individual materials and also when compared to the other combined systems. However, its strain capacity was the greatest .4.3. ShrinkageShrinkage is another important property regarding the dimensional stability of combined systems. Incompatibility due to drying shrinkage causes internal stresses, which might lead to failure at the interface or within the lower strength material. The shrinkage results of the different combined systems are presented in Figs. 7(a)–(e), where the average combined values (Comb) are plotted with values of the individual materials.The results indicate that long moist curing (3 months) significantly reduces the shrinkage of the control concrete even when exposed to the hot dry environment. Most of the shrinkage strains developed within the first 2 weeks, after which it levelled off to show an overall low shrinkage value at the age of 28 days. In contrast to the modulus results, the EP/concrete system (Fig. 7(e)) showed similar behaviour to that of the parent concrete indicating compatibility of shrinkage behaviour. The PMC repair mortars usually incorporate expanding additives whichreduce the shrinkage at early age. This can be seen in Fig. 7(d), where the PMC/ concrete system showed low shrinkage values within the first 10 days, after which the rate of shrinkage development was relatively higher than that of the parent concrete.Incompatibility of shrinkage can be seen clearly from comparing the combined systems with the cementitious repair mortars. Table 5 gives the 28-day shrinkage for the individual materials (S c, S m) together with the average combined measured values (S0) and as calculated from Eq. (9). It should be noted that the values of E in Eq. (2) should strictly be effective values to account for creep. In the present analysis, the difference in creep between the repair material and the parent concrete was neglected. Also, it was assumed that no moisture transfer occurred across the interface since the fractured surface of the substrate was sprayed with water prior to the repair materials being cast.Table 5. Shrinkage strain at 28 days (Microstrains)The highest differential shrinkage was found with the OPC/concrete system. The FA and SF combined systems showed similar behaviour to that of the OPC/ concrete.Similar to the modulus results, the combined shrinkage values (calculated and measured) agree to within 10%, confirming the validity of the combined model proposed and the small influence of Poisson's ratio as considered in the derivation of Eq. (7).4.4. Transport propertiesThe transport properties are of great importance when considering the durability of the repair system. The combined specimens were conditioned and tested in a similar manner to the individual materials used in [11] following the procedure described in [12and 13], which involve the removal of the evaporable water to eliminate the effect of moisture on the measured transport properties. The effect of differential shrinkage on the intrinsic coefficient of oxygen permeability of the combined systems is presented , whereas gives the coefficient of oxygen diffusionresults.By comparing the results of the combined systems, it can be seen that the OPC/ concrete system exhibited the highest permeability value whereas the lowest value was found with the EP/concrete system. This trend is similar to that obtained from the shrinkage results. In fact the permeability of the OPC/concrete and the FA/ concrete systems were about one order of magnitude higher than the individual materials (OPC, FA mortars and parent concrete).The results of oxygen diffusion agree with those obtained from the permeability results. The shrinkage compatibility of the EP/concrete system reduces the diffusion value to be similar to that of the parent concrete.The performance of the PMC/concrete system was adequate when compared with the EP/concrete system.In general the trend of the transport properties of the combined specimens appears to be associated with the differential shrinkage found with the different systems. This would be expected since any weakening at the interface, and consequent increase in permeability and diffusion, would be greater for a higher differential shrinkage. show the coefficients of permeability and diffusion plotted against the relative shrinkage (S m/S c) for the tested combined systems. The general linear relationships obtained indicate that higher differential shrinkage results in higher transport properties and therefore lower resistance to the penetration of harmful substances from aggressive environments.5. DiscussionCompatibility of concrete and repair materials involves matching of different properties between the two systems, as mentioned earlier. Dimensional stability under load application (modulus) was one of the issues considered in this study for the different systems investigated.Mismatch in the modulus of elasticity becomes of great concern in repairs when the applied load is parallel to the bond line in a combined system. The material with the lower modulus deforms more and, therefore, transfers the load, through the interface, to the higher modulus material [14]. If the transferred load exceeds theload-carrying capacity of the material or the bond at the interface, fracture occurs. For the design of an efficient repair,it has been recommended that the repair material should have greater modulus (>30%) than the concrete substrate [15]. Within the different repair mortars used in the study the cementitious mortars provided almost similar moduli values to that of the parent concrete,whereas the mismatch can be seen clearly with the epoxy (polymer) mortar used ( Table 3). Due to the high bond strength of epoxy mortars [16], it forces the concrete to deform more under load application ( Fig. 5and Fig. 6), leading to an early concrete fracture and consequently failure of the combined system.Drying shrinkage was the other parameter used to study the dimensional stability in repair systems. It is mainly influenced by the composition of the materials and the surrounding environments, and achieves a great part of its ultimate value at early ages considering the small size of the samples tested [17]. Larger specimens with a higher volume-to-surface ratio will definitely take more time to shrink. As the fresh repair material tends to shrink, the parent concrete(relatively old) restrains it. The differential movements cause tensile stresses in the repair mortar balanced by compressive stresses within the concrete.Creep in such a situation is an advantage, as it releases part of these stresses. As shrinkage proceeds, the stresses accumulate, which might cause cracks and failure if exceeded the tensile capacity of the repair material or the bond strength at the interface.In contrast to the modulus results, the shrinkage incompatibility is more associated with the cementitious mortars, which reduces sharply with the use of PMC to reach minimum for polymer (EP) mortars. Similar trend of results was found with the transport properties of the different systems, suggesting their dependence on the dimensional stability of combined systems. A general correlation appears to exist between transport properties and differential shrinkage.In general, the results obtained in this investigation indicate that in spite of the superior properties of the epoxy mortar, its compatibility with concrete is mainly affected by the low modulus. The high shrinkage of the cementitious mortars, especially when exposed to hot dry environments limits their compatibility. The most appropriate performance was obtained for the PMC mortar, which showed adequate compatibility in modulus and shrinkage with improved engineering and transportproperties.6. ConclusionsFor the repair materials used in this study and stored under a hot-dry environment, the conclusions can be summarised as follows:1. High shrinkage strains of the cementitious repair mortars affected their compatibility with concrete,and increased indirectly the permeability at the interface of the combined system by one order of magnitude.2. The mismatch in modulus of elasticity between concrete and the epoxy mortar used in the study reduced the load carrying capacity of the combined system.3. Transport properties (namely permeability and diffusion) correlated fairly well with differential shrinkage of the repair material and parent concrete.4. The PMC repair mortar showed the most appropriate properties in terms of dimensional stability with concrete due to similar elastic modulus and low shrinkage strains when compared to the parent concrete.Future research should investigate the dimensional compatibility, including creep and autogenous shrinkage, of repair materials with microstructural studies of the interface and transition zoneCopyright © 1996 Published by Elsevier Science Ltd.K. E. Hassan,, J. J. Brooks and L. Al-Alawi中文翻译1在干燥环境下砂浆和混凝土修复的兼容性摘要:在民用工程领域中,建筑物的加固、维护和修理将被更多得关注到。
土木工程混凝土强度中英文对照外文翻译文献

<文献翻译一:原文>Strength of Concrete in Slabs, Investigates along Direction of Concreting ABSTRACTIn theory of concrete it is assumed that concrete composites are isotropic on a macro scale. For example, it is assumed that a floor slab’s or a beam’s streng th is identical in all directions and its nonhomogeneity is random. Hence neither calculations of the load-bearing capacity of structural components nor the techniques of investigating concrete in structure in situ take into account to a sufficient degree the fact that the assumption about concrete isotropy is overly optimistic. The present research shows that variation in concrete strength along the direction of concreting has not only a qualitative effect (as is commonly believed), but also a significant quantitative effect. This indicates that concrete is a composite which has not been fully understood yet. The paper presents evaluations of ordinary concrete (OC) homogeneity along component thickness along the direction of concreting. The ultrasonic method and modified exponential heads with a point contact with concrete were used in the investigations [1-3].Keywords: Concrete; Compressive Strength of Concrete; Non-Destructive1. IntroductionIn a building structure there are components which are expected to have special properties but not necessarily in the whole cross section. Components under bending, such as beams and floor slabs are generally compressed in their upper zone and the concrete’s compressive strength is vital mainly in this zone. The components are usually moulded in the same position in which they later remain in service, i.e. with their upper zone under compression. Concrete in the upper zone is expected to be slightly weaker than in the lower zone, but it is unclear how much weaker [4,5]. Also flooring slabs in production halls are most exposed to abrasion and impact loads in their upper zone which is not their strongest part. It is known from practice that industrial floors belong to the most often damaged building components.When reinforced concrete beams or floor slabs are to be tested they can be accessed only from their undersides and so only the bottom parts are tested and on this basis conclusions are drawn about the strength of the concrete in the whole cross section, including in the compressed upper zone. Thus a question arises: how large are the errors committed in this kind of investigations?In order to answer the above and other questions, tests of the strength of concrete in various structural components, especially in horizontally concreted slabs, were carried out. The variation of strength along the thickness of the components was analyzed.2. Research SignificanceThe research results presented in the paper show that the compressive strength of concrete in horizontally formed structural elements varies along their thickness. In the top zone the strength is by 25% - 30% lower than the strength in the middle zone, and it can be by as much as 100% lower than the strength in the bottom zone. The observations are based on the results of nondestructive tests carried out on drill cores taken from the structure, and verified by a destructive method. It is interesting to note that despite the great advances in concrete technology, the variation in compressive strength along the thickness of structural elements is characteristic of both old (over 60 years old) concretes and contemporary ordinary concretes.3. Test MethodologyBefore Concrete strength was tested by the ultrasonic method using exponential heads with a point contact with concrete. The detailed specifications of the heads can be found in [2,3]. The heads’ frequency was 40 and 100 kHz and the diameter of their concentrators amounted to 1 mm.In order to determine the real strength distributions in the existing structures, cylindrical cores 80 mm or 114 mm diameter (Figure 2) were drilled from them in the direction of concreting. Then specimens with their height equal to their diameter were cut out of the cores.Ultrasonic measurements were performed on the cores according to the scheme shown in Figure3. Ultrasonic pulses (pings) were passed through in two perpendicular directions I and II in planes spaced every 10 mm. In this way one could determine how ping velocity varied along the core’s height, i.e. along the thickness of the tested component.In both test directions ping pass times were determined and velocities CL were calculated. The velocities from the two directions in a tested measurement plane were averaged.Subsequently, specimens with their height equal to their diameter of 80 mm were cut out of the cores. Aver-age ultrasonic pulse velocity CL for the specimen’s central zone was correlated with fatigue strength fc determined by destructive tests carried out in a strength tester.For the different concretes different correlation curves with a linear, exponential or power equation were obtained. Exemplary correlation curve equations are given below:Lc c L c C f L f C f 38.1exp 0951.01.003.56705.232621.4=⋅=-⨯=where:fc —the compressive strength of concrete MPa,CL —ping velocity km/s.The determined correlation curve was used to calculate the strength of concrete in each tested core cross section and the results are presented in the form of graphs illustrating concrete strength distribution along the thickness of the tested component. 4. Investigation of Concrete in Industrial FloorsAfter Floor in sugar factory’s raw materials storage hall Concrete in an industrial floor must have particularly good characteristics in the top layer. Since it was to be loaded with warehouse trucks and stored sugar beets and frequently washed the investigated concrete floor (built in 1944) was designed as consisting of a 150 mm thick underlay and a 50 mm thick surface layer and made of concrete with a strength of 20 MPa (concrete A).As part of the investigations eight cores, each 80 mm in diameter, were drilled from the floor. The investigations showed significant departures from the design. The concrete subfloor’s thickness varied from 40 to 150 mm. The surface layer was not made of concrete, but of cement mortar with sand used as the aggregate. Also the thickness of this layer was uneven, varying from 40 to 122mm. After the ultrasonic tests specimens with their height equal to their diameter of 80 mm were cut out of the cores. Two scaling curves: one for the surface layer and the other for the bottom concrete layer were determined.A characteristic concrete compressive strength distribution along the floor’s thickness is shown in Figure 4.Strength in the upper zone is much lower than in thelower zone: ranging from 4.7 to 9.8 MPa for the mortar and from 13.9 to 29.0 MPa for the concrete layer. The very low strength of the upper layer of mortar is the result of strong porosity caused by air bubbles escaping upwards during the vibration of concrete. Figure 5 shows t he specimen’s porous top surface.Floor in warehouse hall with forklift truck transport The floor was built in 1998. Cellular concrete was used as for the underlay and the 150 mm thick surface layer was made of ordinary concrete with fibre (steel wires) reinforcement (concrete B). Cores 80 mm high and 80 mm in diameter were drilled from the surface layer. Ultrasonic measurements and destructive tests were performed as described above. Also the test results were handled in a similar way. An exemplary strength distribution along the floor’s thickness is shown in Figure 6.5. ConclusionsTests of ordinary concretes show unexpectedly greatly reduced strength in the upper zone of horizontally moulded structural components. This is to a large degree due to the vibration of concrete as a result of which coarse aggregate displaces downwards making the lower layers more compact while air moves upwards aerating the upper layers and thereby increasing their porosity. The increase in the concrete’s porosity results in a large drop in its compressive strength. Thanks to the use of the ultrasonic method and probes with exponential concentrators it could be demonstrated how the compressive strength of ordinary concrete is distributed along the thickness of structural components in building structures. It became apparent that the reduction in compressive strength in the compressed zone of structural components under bending and in industrial concrete floors can be very large (amounting to as much as 50% of the strength of t he slab’s lower zone). Therefore this phenomenon should be taken into account at the stage of calculating slabs, reinforced concrete beams and industrial floors [6].The results of the presented investigations apply to ordinary concretes (OC) which are increasingly supplanted by self-compacting concretes (SCC) and high-performance concretes (HPC). Since no intensive vibration is required to mould structures from such concretes one can expect that they are much more homogenous along their thickness [7]. This will be known once the ongoing experimental research is completed.Bohdan StawiskiStrength of Concrete in Slabs, Investigates along Direction of Concreting[D]Institute of Building Engineering, Wroclaw University of Technology Wybrzeze Wyspianskiego, Wroclaw, Poland Received October 15, 2011; revised November 21, 2011; accepted November 30, 2011<文献翻译一:译文>混凝土强度与混凝土浇筑方向关系的研究摘要从理论上看,假设混凝土复合材料是各项同性的从宏观尺度上讲。
土木工程毕业论文中英文翻译

外文翻译班级:xxx学号:xxx姓名:xxx一、外文原文:Structural Systems to resist lateral loadsCommonly Used structural SystemsWith loads measured in tens of thousands kips, there is little room in the design of high-rise buildings for excessively complex thoughts. Indeed, the better high-rise buildings carry the universal traits of simplicity of thought and clarity of expression.It does not follow that there is no room for grand thoughts. Indeed, it is with such grand thoughts that the new family of high-rise buildings has evolved. Perhaps more important, the new concepts of but a few years ago have become commonplace in today’ s technology.Omitting some concepts that are related strictly to the materials of construction, the most commonly used structural systems used in high-rise buildings can be categorized as follows:1.Moment-resisting frames.2.Braced frames, including eccentrically braced frames.3.Shear walls, including steel plate shear walls.4.Tube-in-tube structures.5.Core-interactive structures.6.Cellular or bundled-tube systems.Particularly with the recent trend toward more complex forms, but in response also to the need for increased stiffness to resist the forces from wind and earthquake, most high-rise buildings have structural systems built up of combinations of frames, braced bents, shear walls, and related systems. Further, for the taller buildings, the majorities are composed of interactive elements in three-dimensional arrays.The method of combining these elements is the very essence of the design process for high-rise buildings. These combinations need evolve in response to environmental, functional, and cost considerations so as to provide efficient structures that provoke the architectural development to new heights. This is not to say that imaginative structural design can create great architecture. To the contrary, many examples of fine architecture have been created with only moderate support from thestructural engineer, while only fine structure, not great architecture, can be developed without the genius and the leadership of a talented architect. In any event, the best of both is needed to formulate a truly extraordinary design of a high-rise building.While comprehensive discussions of these seven systems are generally available in the literature, further discussion is warranted here .The essence of the design process is distributed throughout the discussion.Moment-Resisting FramesPerhaps the most commonly used system in low-to medium-rise buildings, the moment-resisting frame, is characterized by linear horizontal and vertical members connected essentially rigidly at their joints. Such frames are used as a stand-alone system or in combination with other systems so as to provide the needed resistance to horizontal loads. In the taller of high-rise buildings, the system is likely to be found inappropriate for a stand-alone system, this because of the difficulty in mobilizing sufficient stiffness under lateral forces.Analysis can be accomplished by STRESS, STRUDL, or a host of other appropriate computer programs; analysis by the so-called portal method of the cantilever method has no place in today’s technology.Because of the intrinsic flexibility of the column/girder intersection, and because preliminary designs should aim to highlight weaknesses of systems, it is not unusual to use center-to-center dimensions for the frame in the preliminary analysis. Of course, in the latter phases of design, a realistic appraisal in-joint deformation is essential.Braced Frame sThe braced frame, intrinsically stiffer than the moment –resisting frame, finds also greater application to higher-rise buildings. The system is characterized by linear horizontal, vertical, and diagonal members, connected simply or rigidly at their joints. It is used commonly in conjunction with other systems for taller buildings and as a stand-alone system in low-to medium-rise buildings.While the use of structural steel in braced frames is common, concrete frames are more likely to be of the larger-scale variety.Of special interest in areas of high seismicity is the use of the eccentric braced frame.Again, analysis can be by STRESS, STRUDL, or any one of a series of two –or three dimensional analysis computer programs. And again, center-to-center dimensions are used commonly in the preliminary analysis.Shear wallsThe shear wall is yet another step forward along a progression of ever-stiffer structural systems. The system is characterized by relatively thin, generally (but not always) concrete elements that provide both structural strength and separation between building functions.In high-rise buildings, shear wall systems tend to have a relatively high aspect ratio, that is, their height tends to be large compared to their width. Lacking tension in the foundation system, any structural element is limited in its ability to resist overturning moment by the width of the system and by the gravity load supported by the element. Limited to a narrowoverturning, One obvious use of the system, which does have the needed width, is in the exterior walls of building, where the requirement for windows is kept small.Structural steel shear walls, generally stiffened against buckling by a concrete overlay, have found application where shear loads are high. The system, intrinsically more economical than steel bracing, is particularly effective in carrying shear loads down through the taller floors in the areas immediately above grade. The system has the further advantage of having high ductility a feature of particular importance in areas of high seismicity.The analysis of shear wall systems is made complex because of the inevitable presence of large openings through these walls. Preliminary analysis can be by truss-analogy, by the finite element method, or by making use of a proprietary computer program designed to consider the interaction, or coupling, of shear walls.Framed or Braced TubesThe concept of the framed or braced or braced tube erupted into the technology with the IBM Building in Pittsburgh, but was followed immediately with the twin 110-story towers of the World Trade Center, New York and a number of other buildings .The system is characterized by three –dimensional frames, braced frames, or shear walls, forming a closed surface more or less cylindrical in nature, but of nearly any plan configuration. Because those columns that resist lateral forces are placed as far as possible from the cancroids of the system, the overall moment of inertia is increased and stiffness is very high.The analysis of tubular structures is done using three-dimensional concepts, or by two- dimensional analogy, where possible, whichever method is used, it must be capable of accounting for the effects of shear lag.The presence of shear lag, detected first in aircraft structures, is a serious limitation in the stiffness of framed tubes. The concept has limited recent applications of framed tubes to the shear of 60 stories. Designers have developed various techniques for reducing the effects of shear lag, most noticeably the use of belt trusses. This system finds application in buildings perhaps 40stories and higher. However, except for possible aesthetic considerations, belt trusses interfere with nearly every building function associated with the outside wall; the trusses are placed often at mechanical floors, mush to the disapproval of the designers of the mechanical systems. Nevertheless, as a cost-effective structural system, the belt truss works well and will likely find continued approval from designers. Numerous studies have sought to optimize the location of these trusses, with the optimum location very dependent on the number of trusses provided. Experience would indicate, however, that the location of these trusses is provided by the optimization of mechanical systems and by aesthetic considerations, as the economics of the structural system is not highly sensitive to belt truss location.Tube-in-Tube StructuresThe tubular framing system mobilizes every column in the exterior wall in resisting over-turning and shearing forces. The term‘tube-in-tube’is largely self-explanatory in that a second ring of columns, the ring surrounding the central service core of the building, is used as an inner framed or braced tube. The purpose of the second tube is to increase resistance to overturning and to increase lateral stiffness. The tubes need not be of the same character; that is, one tube could be framed, while the other could be braced.In considering this system, is important to understand clearly the difference between the shear and the flexural components of deflection, the terms being taken from beam analogy. In a framed tube, the shear component of deflection is associated with the bending deformation of columns and girders (i.e, the webs of the framed tube) while the flexural component is associated with the axial shortening and lengthening of columns (i.e, the flanges of the framed tube). In a braced tube, the shear component of deflection is associated with the axial deformation of diagonals while the flexural component of deflection is associated with the axial shortening and lengthening of columns.Following beam analogy, if plane surfaces remain plane (i.e, the floor slabs),then axial stresses in the columns of the outer tube, being farther form the neutral axis, will be substantially larger than the axial stresses in the inner tube. However, in the tube-in-tube design, when optimized, the axial stresses in the inner ring of columns may be as high, or even higher, than the axial stresses in the outer ring. This seeming anomaly is associated with differences in the shearing component of stiffness between the two systems. This is easiest to under-stand where the inner tube is conceived as a braced (i.e, shear-stiff) tube while the outer tube is conceived as a framed (i.e, shear-flexible) tube.Core Interactive StructuresCore interactive structures are a special case of a tube-in-tube wherein the two tubes are coupled together with some form of three-dimensional space frame. Indeed, the system is used often wherein the shear stiffness of the outer tube is zero. The United States Steel Building, Pittsburgh, illustrates the system very well. Here, the inner tube is a braced frame, the outer tube has no shear stiffness, and the two systems are coupled if they were considered as systems passing in a straight line from the “hat” structure. Note that the exterior columns would be improperly modeled if they were considered as systems passing in a straight line from the “hat” to the foundations; these columns are perhaps 15% sti ffer as they follow the elastic curve of the braced core. Note also that the axial forces associated with the lateral forces in the inner columns change from tension to compression over the height of the tube, with the inflection point at about 5/8 of the height of the tube. The outer columns, of course, carry the same axial force under lateral load for the full height of the columns because the columns because the shear stiffness of the system is close to zero.The space structures of outrigger girders or trusses, that connect the inner tube to the outer tube, are located often at several levels in the building. The AT&T headquarters is an example of an astonishing array of interactive elements:1.The structural system is 94 ft (28.6m) wide, 196ft(59.7m) long, and 601ft (183.3m) high.2.Two inner tubes are provided, each 31ft(9.4m) by 40 ft (12.2m), centered 90 ft (27.4m) apart in the long direction of thebuilding.3.The inner tubes are braced in the short direction, but with zero shear stiffness in the long direction.4. A single outer tube is supplied, which encircles the building perimeter.5.The outer tube is a moment-resisting frame, but with zero shear stiffness for the center50ft (15.2m) of each of the longsides.6. A space-truss hat structure is provided at the top of the building.7. A similar space truss is located near the bottom of the building8.The entire assembly is laterally supported at the base on twin steel-plate tubes, because the shear stiffness of the outertube goes to zero at the base of the building.Cellular structuresA classic example of a cellular structure is the Sears Tower, Chicago, a bundled tube structure of nine separate tubes. While the Sears Tower contains nine nearly identical tubes, the basic structural system has special application for buildings of irregular shape, as the several tubes need not be similar in plan shape, It is not uncommon that some of the individual tubes one of the strengths and one of the weaknesses of the system.This special weakness of this system, particularly in framed tubes, has to do with the concept of differential column shortening. The shortening of a column under load is given by the expression△=ΣfL/EFor buildings of 12 ft (3.66m) floor-to-floor distances and an average compressive stress of 15 ksi (138MPa), the shortening of a column under load is 15 (12)(12)/29,000 or 0.074in (1.9mm) per story. At 50 stories, the column will have shortened to 3.7 in. (94mm) less than its unstressed length. Where one cell of a bundled tube system is, say, 50stories high and an adjacent cell is, say, 100stories high, those columns near the boundary between .the two systems need to have this differential deflection reconciled.Major structural work has been found to be needed at such locations. In at least one building, the Rialto Project,Melbourne, the structural engineer found it necessary to vertically pre-stress the lower height columns so as to reconcile the differential deflections of columns in close proximity with the post-tensioning of the shorter column simulating the weight to be added on to adjacent, higher columns.二、原文翻译:抗侧向荷载的结构体系常用的结构体系若已测出荷载量达数千万磅重,那么在高层建筑设计中就没有多少可以进行极其复杂的构思余地了。
土木工程外文文献翻译(含中英文)

Experimental research on seismic behavior of abnormal jointin reinforced concrete frameAbstract :Based on nine plane abnormal joint s , one space abnormal joint experiment and a p seudo dynamic test of a powerplant model , the work mechanism and the hysteretic characteristic of abnormal joint are put to analysis in this paper. A conception of minor core determined by the small beam and small column , and a conclusion that the shear capacity of ab2normal joint depends on minor core are put forward in this paper. This paper also analyzes the effect s of axial compres2 sion , horizontal stirrup s and section variation of beam and column on the shear behavior of abnormal joint . Finally , the formula of shear capacity for abnormal joint in reinforced concrete f rame is provided.Key words : abnormal j oint ; minor core ; seismic behavior ; shear ca paci t yCLC number :TU375. 4 ; TU317. 1 Document code :A Article ID :100627930 (2006) 022*******1 Int roductionFor reinforced concrete f rame st ructure , t he joint is a key component . It is subjected to axialcomp ression , bending moment and shear force. The key is whet her the joint has enough shear capaci2ty. The Chinese Code f or S eismic Desi gn of B ui l di ngs ( GB5001122001) adopt s the following formulato calculate t he shear capacity of the reinforced concrete f rame joint .V j = 1. 1ηj f t b j h j + 0. 05ηj Nb jb c+ f yv A svjh b0 - a′ss(1)Where V j = design value of t he seismic shear capacity of the joint core section ;ηj = influential coefficient of t he orthogonal beam to the column ;f t = design value of concrete tensile st rength ;b j = effective widt h of the joint core section ;h j = dept h of the joint core section , Which can be adopted as t he depth of the column section int he verification direction ;N = design value of axial compression at t he bot tom of upper column wit h considering the combi2 nation of the eart hquake action , When N > 015 f c b c h c , let N = 0. 5 f c b c h c ;b c = widt h of t he column section ;f yv = design value of t he stirrup tensile st rengt h ;A svj = total stirrup area in a set making up one layer ;h b0 = effective dept h of t he beam.If t he dept h of two beams at the side of t he joint is unequal , h b0 = t he average depth of two beams.a′s = distance f rom the cent roid of the compression beam steel bar to the ext reme concrete fiber . s = distance of t he stirrup .Eq. 1 is based on t he formula in t he previous seismiccode[1 ] and some modifications made eavlicr and it is suit2able to the normal joint of reinforced concrete f rame , butnot to t he abnormal one which has large different in t hesection of t he upper column and lower one (3 600 mm and1 200 mm) , lef t beam and right beam (1 800 mm and 1200 mm) . The shear capacity of abnormal joint s calculat2ed by Eq. 1 may cause some unsafe result s. A type of ab2normal joint which of ten exist s in t he power plant st ruc2t ure is discussed ( see Fig. 1) , and it s behavior was st ud2ied based on t he experiment in t his paper2 Experimental workAccording to the above problem , and t he experiment of plane abnormal joint s and space abnormal joint , a p seudo dynamic test of space model of power plant st ruct ure was carried out . The aim of t hisst udy is to set up a shear force formula and to discuss seismic behavior s of t he joint s.According to the characteristic of t he power plant st ruct ure , nine abnormal joint s and one space abnormal joint were designed in t he experiment . The scale of the model s is one2fif t h. Tab. 1 and Tab.2 show t he dimensions and reinforcement detail s of t he specimens.Fig. 2 shows the typical const ruction drawing of t he specimen. Fig. 3 shows the loading set up . These specimens are subjected to low2cyclic loading , the loading process of which is cont rolled by force and displacement , t he preceding yield loading by force and subsequent yield by t he displacement .The shear deformation of the joint core , t he st rain of the longit udinal steel and t he stirrup are main measuring items.3 Analysis of test result s3. 1 Main resultsTab. 3 shows t he main result s of t he experiment .3. 2 Failure process of specimenBased on t he experiment , t he process of t he specimens’failure includes four stages , namely , t he initial cracking , t he t horough cracking , the ultimate stage and t he failure stage.(1) Initial cracking stageWhen t he first diagonal crack appears along t he diagonal direction in t he core af ter loading , it s widt h is about 0. 1mm , which is named initial cracking stage of joint core. Before t he initial cracking stage , t he joint remains elastic performance , and the variety of stiff ness is not very obvious on t hep2Δcurve. At t his stage concrete bear s most of the core shear force while stirrup bears few. At t he timewhen t he initial crack occur s , t he st ress of t he stirrup at t he crack increase sharply and t he st rain is a2bout 200 ×10 - 6 —300 ×10 - 6 . The shear deformation of t he core at t his stage is very small (less than 1×10 - 3 radian ,generally between 0. 4 ×10 - 3 and 0. 8 ×10 - 3 radian) .(2) Thorough cracking stageWit h the load increasing following t he initial cracking stage , the second and t hird crossing diago2 nal cracks will appear at t he core. The core is cut into some small rhombus pieces which will become at least one main inclined crack across t he core diagonal . The widt h of cracks enlarges obviously , andt he wider ones are generally about 0. 5mm , which is named core t horough cracking stage. The st ress of stirrup increases obviously , and the stirrup in t he middle of t he core is near to yielding or has yiel2 ded. The joint core shows nonlinear property on t he p2Δcurve , and it enter s elastic2plastic stage. Theload at t horough cracking stage is about 80 % —90 % load.(3) Ultimate stageAt t his stage , t he widt h of t he cracks is about 1mm or more and some new cracks continue to oc2 cur . The shear deformation at t he core is much larger and concrete begins to collap se. Af ter several cyclic loading , the force reaches the maximum value , which is called ultimate stage. The load increase is due to t he enhancing of the concrete aggregate mechanical f riction between cracks. At t he same timet he st ress of stirrup increases gradually. On t he one hand stirrup resist s t he horizontal shear , and on t he ot her hand the confinement effect to t he expanding compression concrete st rengthens continuous2ly , which can also improve t he shear capacity of diagonal compression bar mechanism.(4) Failure stageAs the load circulated , concrete in t he core began to collap se , and t he deformation increased sharply , while the capacity began to drop . It was found t hat t he slip of reinforcement in t he beam wasvery serious in t he experiment . Wit h t he load and it s circulation time increasing , t he zoon wit houtbond gradually permeated towards t he internal core , enhancing t he burden of t he diagonal compressionbar mechanism and accelerates the compression failure of concrete. Fig. 4 shows t he p hotos of typical damaged joint s.A p seudo dynamic test of space model ofpower plant st ruct ure was carried out to researcht he working behavior of t he abnormal joint s in re2al st ructure and the seismic behavior of st ructure.Fig. 5 shows the p hoto of model .The test includes two step s. The fir st is thep seudo dynamic test . At t his step , El2Cent rowave is inp ut and the peak acceleration variesf rom 50 gal to 1 200 gal . The seismic response is measured. The second is t he p seudo static test . Theloading can’t stop until t he model fail s.Fig. 7 Minor coreThe experiment shows t hat t he dist ribution and development of t hecrack is influenced by t he rest rictive effect of the ort hogonal beam , andt he crack of joint core mainly dist ributes under t he orthogonal beam( see Fig. 6) , which is different f rom t he result of t he plane joint test ,but similar to J 4210.3. 3 Analysis of test results3. 3. 1 Mechanical analysisIn t he experiment , t he location of the initial crack of t he exteriorjoint and the crushed position of concrete both appear in the middle oft he joint core , and t he position is near t he centerline of t he upper col2umn. The initial crack and crushed position of t he concrete of the interior joint both appear in t he mi2 nor core ( see Fig. 4 ,Fig. 7) . For interior abnormal joint t he crack doesn’t appear or develop in t he ma2j or core out side of the mi nor core until t horough cracking takes place , while t he crack seldom appearsin t he shadow region ( see Fig. 7) as the joint fail s. Therefore , for abnormal joint , t he shear capacity oft he joint core depends on t he properties of t he mi nor core , namely , on t he st rengt h grades of concrete ,t he size and the reinforcement of t he mi nor core , get t he effect of t he maj or core dimension can’t be neglected.Mechanical effect s are t he same will that of t he normal joint , when t he forces t ransfer to t he mi2 nor core t hrough column and beam and reinforcement bar . Therefore , t he working mechanisms of nor2mal joint , including t russ mechanism , diagonal compression bar mechanism and rest rictive mechanismof stirrup , are also suitable for mi nor core of t he abnormal joint , but their working characteristic is not symmet rical when the load rever ses. Fig. 8 illust rates t he working mechanism of t he abnormal joint .When t he load t ransfer to mi nor core , t he diagonal compression bar area of mi nor core is biggert han normal joint core2composed by small column and small beam of abnormal joint , which is due to t he compressive st ress diff usion of concrete compressive region of the beam and column , while at t hesame time t he compression carried by the diagonal compression bar becomes large. Because t he main part of bond force of column and beam is added to t he diagonal comp ression bar but cont rasting wit h t he increased area of diagonal compression bar , t he increased action is small . The region in the maj orcore but out of the mi nor core has less st ress dist ribution and fewer cracks. The region can confine t heexpansion of t he concrete of t he mi nor core diagonal compression bar concrete , which enhances t he concrete compressive st rengt h of mi nor core diagonal compression bar .Making t he mi nor core as st udy element , the area increment of concrete diagonal compression barin mi nor core is related to t he st ress diff usion of t he beam and column compressive region. The magni2t ude of diff usion area is related to height difference of t he beam sections and column sections. Name2ly , it is related to t he size of mi nor core section and maj or core section. Thus , the increased shearst rengt h magnit ude caused by mi nor core rest rictive effect on maj or core can be measured quantitative2ly by t he ratio of maj or core area to mi nor core area. And it al so can be expressed that t he rest rictive effect is quantitatively related to t he ratio. Obviously , t he bigger t he ratio is and t he st ronger t he con2finement is , t he st ronger t he bearing capacity is.The region in the maj or core but under the mi nor core still need stirrup bar because of t he hori2 zontal force t ransferred by bigger beam bar . But force is small .3. 3. 2 load2displacement curves analysisFig. 9 shows t he typical load2displacement curves at t he beam end of t he exterior and interiorjoint . The figure showing t hat t he rigidity of t he specimens almo st doesn’t degenerate when t he initialcrack appear s in t he core , and a turning point can be found at t he curve but it isn’t very obvious. Wit ht he crack developing , an obvious t urning point can be found at t he curve , and at t his time , t he speci2men yields. Then t he load can increase f urt her , but it can’t increase too much f rom yielding load to ultimate load. When t he concrete at t he core collap ses and the plastic hinge occured at t he beamend ,t he load begins to decrease rat her t han increase.The ductility coefficient of two kinds of joint s is basically more than 3 (except for J 3 - 9) . But it should be noted t hat the design of specimens is based on the principle of joint core failure. The ratio of reinforcement of beam and column tends to be lower t han practical project s. If t he ratio is larger , t he failure of joint is probably prior to t hat of beam and column , so t he hysteretic curve reflect s t he ductil ity property of joint core.Joint experiment should be a subst ruct ure test (or a test of composite body of beams and col2 umns) . So t he load2displacement curves at t he beam end should be a general reflection of t he joint be2havior work as a subst ruct ure. Providing t hat the joint core fails af ter t he yield of beam and column (especially for beam) , t he load2displacement curves at t he beam end is plump , so the principle of “st rong col umn and weak beam , st ron ger j oi nt" should be ensured which conforms to t he seismic re2sistant principle.The experiment shows t hat t he stiff ness of joint core is large. Before the joint reaches ultimatestage , t he stiff ness of joint core decreases a little and the irrecoverable residual deformation is very small under alternate loading. When joint core enter s failure stage , t he shear deformation increases sharply , and t he stiff ness of joint core decreases obviously , and t he hysteretic curve appears shrink2 age , which is because of t he cohesive slip of beam reinforcement .3. 4 Influential Factors of Abnormal Joint Shear CapacityThe fir st factor is axial compression. Axial compression can enlarge t he compression area of col2 umn , and increase t he concrete compression area of joint core[124 ] . At t he same time , more shearst ransferred f rom beam steel to t he edge of joint core concrete will add to t he diagonal compression bar ,which decreases t he edge shear t hat leads to the crack of joint core concrete. So t he existence of axial comp ression cont ributes to imp roving t he capacity of initial cracks at joint core.The effect of axial compression on t horough cracking load and ultimate load isn’t very obvious[1 ] . The reason is t hat cont rasting wit h no axial compression , the accumulated damage effect of joint coreunder rever sed loading wit h axial compression is larger . Alt hough axial compression can improve t heshear st rengt h of concrete , it increases accumulated damage effect which leads to a decrease of the ad2vantage of axial compression. Therefore t he effect of axial compression on t horough cracking loadandultimate load is not very obvious.Hence , considering the lack of test data of abnormal joint , t he shear capacity formula of abnormal joint adopt 0. 05 nf c b j h j to calculate the effect of axial compression , which is based on the result s of t his experiment and referenced to t he experimental st udy and statistical analysis of Meinheit and J irsa ,et [5 ] .The second factor is horizontal stirrup . Horizontal stirrup has no effect on t he initial crackingshear of abnormal joint , while greatly improves t he t horough cracking shear . Af ter crack appeared , t he stirrup begins to resist t he shear and confines t he expansion of concrete[ 6 ] . This experiment showst hat t he st ress of stirrup s in each layer is not equal . When the joint fail s , t he stirrup s don’t yield simultaneous. Fig. 10 shows t he change of st ress dist ribution of stirrup s along core height wit h t he loadincreasing. Through analyzing test result s , it can be known t hat 80 percent of the height at the joint core can yield.The last factor is the change of sec2tion size of t he beam and column. Thesection change decreases t he initial crack2ing load about 30 p resent of abnormaljoint and makes t he initial crack appear att he position of joint mi nor core. The rea2son for t his p henomenon is t hat small up2per column section makes t he confinementof mi nor core concrete decrease and t heedge shear increase. But t he section change has lit tle effect on thorough cracking load. Af ter t horoughcracking , the joint enter s ultimate state while the external load can’t increase too much , which is dif2 ferent f rom t he behavior of abnormal joint t hat can carry much shear af ter thorough cracking.3. 5 Shear force formula of abnormal jointAs a part of f rame , t he design of joint shall meet t he requirement s of the f rame st ruct ure design , namely , t he joint design should not damage t he basic performance of t he st ruct ure.According to the principle of st ronger j oi nt , it is necessary for joint to have some safety reserva2 tion. The raised cost for conservational estimation of t he joint bearing capacity is small . But t he con2 servational estimation is very important to t he safety of the f rame st ruct ure. At t horough cracking stage , t he widt h of most cracks is more t han 0. 2 mm , which is bigger than t he suggested limit value in t he concrete design code. Big cracks will influence t he durability of st ruct ure. Hence , the bearing capacity at t horough cracking stage is applied to calculating t he bearing capacity of joint . According to t he analysis of t he working mechanisms of abnormal joint , it could be concludedt hat t he bearing capacity of joint core mainly depends on mi nor core when t he force t ransferred f rommaj or core to mi nor core. All kinds of working mechanisms are suitable to mi nor core element . Thus , a formula for calculating t he shear capacity of abnormal joint can be obtained based on Eq. 1. According to the above analysis of influential factor s of shear capacity of abnormal joint , and ref2 erence to Eq. 1 , a formula for calculating t he shear capacity of reinforced concrete f rame abnormal jointis suggested as followsV j = 0. 1ηjξ1 f c b j h j + 0. 1ηj nξ2 f c b j h j +ξ3 f yv A svj h0 - a′s s(2)Where h0 = effective dept h of small beam section in abnormal joint ;ξ1 = influential coefficient consider2ing mi nor core on working as cont rol element for calculating ;ξ2 = influential coefficient considering effect of axial compression ratio , it s value is 0. 5 , andξ3 = influential coefficient considering t hestir2rup doesn’t yield simultaneous , it s value is 0. 8 , n = N/ f c b c h j .From Fig. 8 , the shear capacity of abnormal joint depends on mi nor core , while maj or core has re2st rictive effect on mi nor core. The effect is related to t he ratio of maj or core area to mi nor core area , so assumingξ1 =αA d A x (3)Where A d = area of abnormal joint maj or core , choosing it as t he value of t he dept h of big beam multiplying t he height of lower column ; A x = area of abnormal joint mi nor core , choosing it as t he value oft he depth of small beam multiplying the height of upper column ; andα= parameter to be defined , it s value is 0. 8 derived f rom t he result s of t he experiment ( see Tab. 4)Then Eq. 2 can be replaced byV j = 0. 1ηjαA d A x f c b j h j + 0. 05ηj n f c b j h j + 0. 8 f yv h0 - a′s s(4)4 ConclusionsThe following conclusions can be drawn f rom t his study.(1) The seismic behavior of abnormal joint in reinforced concrete f rame st ruct ure is poor . Af tert horough cracking , t he joint enter s ultimate state while the external load can’t increase too much , andt he safety reservation of joint isn’t sufficient .(2) The characteristic of bearing load of minor core is similar to that of normal joint , but t he area bearing load is different . The shear capacity depend on t he size , t he st rengt h of concrete and the rein2forcement of mi nor core in abnormal joint . The maj or core has rest rictive effect on mi nor core. (3) Joint experiment should be a subst ruct ure test or a test of composite body of beams and col2 umns. Therefore t he load2displacement curves of t he beam end should be a general reflection of t he joint behavior working as a subst ruct ure. Studies of t he hysteretic curve of subst ruct ure should be based on t he whole st ructure. It is critical to guarantee t he stiff ness and st rengt h of joint core in prac2tice.(4) The formula of shear capacity for abnormal joint in reinforced concrete f rame is provided.References[1 ] TAN GJ iu2ru . The seismic behavior of steel reinforced concrete f rame [M] . Nanjing :Dongnan University Press ,1989 :1572163.[2 ] The research group of reinforcement concrete f rame joint . Shear capacity research of reinforced concrete f rame jointon reversed2cyclic loading[J ] . Journal of Building St ructures , 1983 , (6) :9215.[3 ] PAULA Y T ,PARK R. Joint s reinforced concrete f rames designed for earthquake resistance[ R] . New Zealand :De2partment of civil Engineering , University of Canterbury , Christchurch , 1984.[4 ] FU Jian2ping. Seismic behavior research of reinforced concrete f rame joint with the consideration of axialforce[J ] .Journal of Chongqing Univ , 2000 , (5) :23227.[5 ] MEINHEIT D F ,J IRSA J O. Shear st rength of R/ C beam2column connections [J ] . ACI St ructural Journal , 1993 ,(3) :61271.[6 ] KITA YAMA K, OTANI S ,AO YAMA H. Development of design criteria for RC interior beam2column joints ,de2sign of beam2column joint s for seismic resistance[ R] . SP123 ,ACI ,Det roit , 1991 :61272.[7 ] GB5001122001 ,Code for seismic design of buildings [ S] . Beijing : China Architectural and BuildingPress ,2001.钢筋混凝土框架异型节点抗震性能试验研究摘要:基于8个钢筋混凝土框架异型节点的试验研究,分析了异型框架节点的受力与常规框架节点的异同。
土木工程外文翻译
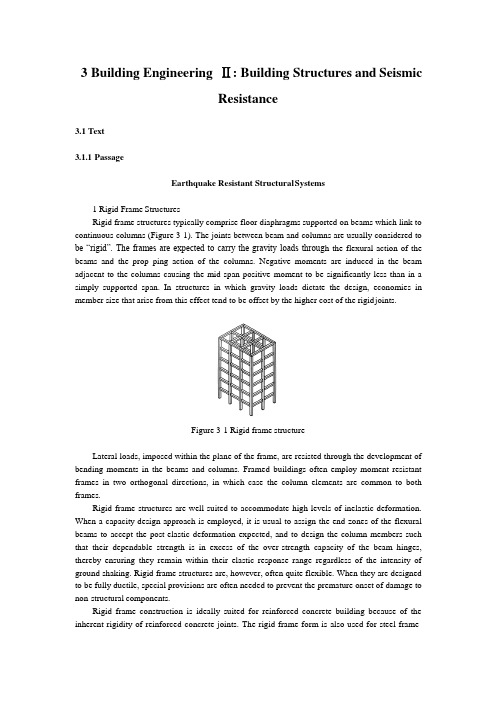
3Building Engineering Ⅱ: Building Structures and SeismicResistanceTextPassageEarthquake Resistant Structural Systems1Rigid Frame StructuresRigid frame structures typically comprise floor diaphragms supported on beams which link to continuous columns (Figure 3-1). The joints between beam and columns are usually considered to be “rigid”. The frames are expected to carry the gravity loads throug h the flexural action of the beams and the prop ping action of the columns. Negative moments are induced in the beam adjacent to the columns causing the mid-span positive moment to be significantly less than in a simply supported span. In structures in which gravity loads dictate the design, economies in member size that arise from this effect tend to be offset by the higher cost of the rigid joints.Figure 3-1 Rigid frame structureLateral loads, imposed within the plane of the frame, are resisted through the development of bending moments in the beams and columns. Framed buildings often employ moment resistant frames in two orthogonal directions, in which case the column elements are common to both frames.Rigid frame structures are well suited to accommodate high levels of inelastic deformation. When a capacity design approach is employed, it is usual to assign the end zones of the flexural beams to accept the post-elastic deformation expected, and to design the column members such that their dependable strength is in excess of the over-strength capacity of the beam hinges, thereby ensuring they remain within their elastic response range regardless of the intensity of ground shaking. Rigid frame structures are, however, often quite flexible. When they are designed to be fully ductile, special provisions are often needed to prevent the premature onset of damage to non-structural components.Rigid frame construction is ideally suited for reinforced concrete building because of the inherent rigidity of reinforced concrete joints. The rigid frame form is also used for steel framebuildings. But moment resistant connections in steel tend to be costly. The sizes of the columns and girders at any level of a rigid-frame are directly influenced by the magnitude of the external shear at that level, and they therefore increase toward the base. Consequently, the design of the floor framing can not be repetitive as it is in some braced frames. A further result is that sometimes it is not possible in the lowest storeys to accommodate the required depth of girder within the normal ceiling space.While rigid frames of a typical scale that serve alone to resist lateral loading have an economic height limit of about 25 storeys, smaller scale rigid frames in the form of a perimeter tube, or typically scaled rigid frames in combination with shear walls or braced bents, can be economic up to much greater heights.2Infilled Frame StructuresInfilled frames (Figure 3-2) are the most usual form of construction for tall buildings of up to 30 storeys in height. Column and girder framing of reinforced concrete, or sometimes steel, is infilled by panels of brickwork, or cast-in-place concrete.Figure 3-2 Infilled frameWhen an infilled frame is subjected to lateral loading, the infill behaves effectively as a strut along its compression diagonal to brace the frame. Because the infills serve also as external walls or internal partitions, the system is an economical way of stiffening and strengthening the structure.The complex interactive behavior of the infill in the frame, and the rather random quality of masonry, had made it difficult to predicate with accuracy the stiffness and strength of an infilled frame. For these reasons, the use of the infills for bracing buildings has mainly been supplementary to the rigid frame action of concrete frames.3Shear WallsA shear wall is a vertical structural element that resists lateral forces in the plane of the wall through shear and bending. The high in plan stiffness and strength of concrete and masonry walls make them ideally suitable for bracing building as shear walls.A shear wall acts as a beam cantilevered out of the ground or foundation9 and, just as with a beam, part of its strength derives from its depth. Figure 3-3 shows two examples of a shear wall, one in a simple one-storey building and another in a multistorey building. In Figure 3-3a, the shear walls are oriented in one direction, so only lateral forces in this direction can be resisted. The roof serves as the horizontal diaphragm and must also be designed to resist the lateral loads and transfer them to the shear walls.a) End shear walls and interior shear wall b) Interior shear walls for bracing in two directionFigure 3-3 Shear wallFigure 3-3a also shows an important aspect of shear walls in particular and vertical elements in general. This is the aspect of symmetry that has a bearing on whether torsional effects will be produced. The shear walls in Figure 3-3a show the shear walls symmetrical in the plane of loading.Figure 3-3b illustrates a common use of shear walls at the interior of a multi-storey building. Because walls enclosing stairways, elevator shafts, and mechanical chases are mostly solid and run the entire height of the building, they are often used for shear walls. Although not as efficient from a strictly structural point of view, interior shear walls do leave the exterior of the building open for windows.Notice that in Figure 3-3b there are shear walls in both directions, which is a more realistic situation because both wind and earthquake forces need to be resisted in both directions. In this diagram, the two shear walls are symmetrical in one direction, but the single shear wall produces a nonsymmetric condition in the other since it is off center. Shear walls do not need to be symmetrical in a building, but symmetry is preferred to avoid torsional effects.If, in low-to medium-rise building, shear walls are combined with frames, it is reasonable to assume that the shear wall attract all the lateral loading so that the frame may be designed for only gravity loading. It is essentially important in shear wall structures to try to plan the wall layout so that the lateral load tensile stresses are suppressed by the gravity load stresses. This allows them to be designed to have only the minimum reinforcement.Since shear walls are generally both stiff and can be inherently robust, it is practical to design them to remain nominally elastic under design intensity loadings, particularly in regions of low or moderate seismicity. Under increased loading intensities, post-elastic deformations will develop within the lower portion of the wall (generally considered to extend over a height of twice the wall length above the foundation support system). Good post-elastic response can be readily achieved within this region of reinforced concrete or masonry shear walls through the provision of adequate confinement of the principal reinforcing steel and the prohibition of lap splices of reinforcing bars.Shear wall structures are generally quite stiff and, as such interstorey drift problems are rare and generally easily contained. The shear wall tends to act as a rigid body rotating about a plastic hinge which forms at the base of the wall. Overall structural deformation is thus a function of the wall rotation. Inter-storey drift problems which do occur are limited to the lower few floors.A major shortcoming with shear walls within buildings is that their size provides internal (or external) access barriers which may contravene the architectural requirements. This problem canbe alleviated by coupling adjacent more slender shear walls so a coupled shear wall structure is formed. The coupling beams then become shear links between the two walls and with careful detailing can provide a very effective, ductile control mechanism (Figure 3-4).Figure 3-4 Coupled shear wall structure4Braced FramesA braced frame is a truss system of the concentric or eccentric type in which the lateral forces are resisted through axial stresses in the members. Just as with a truss, the braced frame depends on diagonal members to provide a load path for lateral forces from each building element to the foundation. Figure 3-5 shows a simple one-storey braced frame. At one end of the building two bays are braced and at the other end only one bay is braced. This building is only braced in one direction and the diagonal member may be either in tension or compression, depending on which way the force is applied.a)Single story braced building b) Multistory braced buildingFigure 3-5 Braced frameFigure 3-5b shows two methods of bracing a multistorey building. A single diagonal compression member in one bay can be used to brace against lateral loads coming from either direction. Alternately, tension diagonals can be used to accomplish the same result, but they must be run both ways to account for the load coming from either direction.Braced framing can be placed on the exterior or interior of a building, and may be placed in one structural bay or several. Obviously, a braced frame can present design problems for windows and doorways, but it is a very efficient and rigid lateral force resisting s ystem.Two major shortcomings of braced systems are that their inclined diagonal orientation oftenconflicts with conventional occupancy use patterns; and secondly they often require careful detailing to avoid large local torsional eccentricities being introduced at the connections with the diagonal brace being offset from the frame node.5Wall-frame StructuresWhen shear walls are combined with rigid frames (Figure 3-6), the walls, which tend to deflect in a flexural configuration, and the frames, which tend to deflect in a shear mode, are constrained to adopt a common shape by the horizontal rigidity of the girders and slabs. As a consequence, the walls and frames interact horizontally, especially at the top, to produce a stiffer and stronger structure. The interacting wall-frame combination is appropriate for buildings in the 40-to-60-storey range, well beyond of rigid frame or shear wall alone.Figure 3-6 Wall-frame structureIn addition, less well-known feature of the wall- frame structure is that, in a carefully “tuned” structure, the shear in the frame can be made approximately uniform over the height, allowing the floor framing to be repetitive.Although the wall-frame structure is usually perceived as a concrete structural form, with shear walls and concrete frames, a steel counterpart using braced frames and steel rigid frames offers similar benefit of horizontal interaction. The braced frames behave with an overall flexural tendency to interact with the shear mode of the rigid frames.6Framed-Tube StructuresThe lateral resistance of framed-tube structures is provided by very stiff moment resisting frames that form a “tube” around the perimeter of the building. The frames c onsist of closely spaced column, 2~4m between centers, joined by deep spandrel girders (Figure 3-7). Although the tube carries all the lateral loading, the gravity load is shared between the tube and interior columns or walls. When lateral loading acts, the perimeter frames aligned in the direction of loading act as the “web” of the massive tube cantilever, and those normal to the direction of the loading act as the “flanges”.Figure 3-7 Frame-tube structureThe close spacing of the columns throughout the height of the structures is usually unacceptable at the entrance level. The columns are therefore merged, or terminated on a transfer beam, a few storeys above the base so that only a few, larger, more widely spaced columns continue to the base. The tube form was developed originally for buildings of rectangular plan; however, for other plan shapes, and has occasionally been used in circular and triangular configurations.The tube is suitable for both steel and reinforced construction and has been used for buildings ranging from 40 to more storeys. The highly repetitive pattern of the frames lends itself to prefabrication in steel, and to the use of rapidly gang forms in concrete, which make for rapid construction.The framed tube has been one of the most significant modern developments in high-rise structural form. It offers a relatively efficiently, easily constructed structure, appropriate for use up to the greatest of heights. Aesthetically, the tube’s externally evident form is regarded with mixed enthusiasm: some praise the logical clearly expressed structure while others criticize the girder-like façade as small-windowed and uninteresting repetitious.The tube structure’s structural efficiency, although hi gh, still leaves scope for improvement because the “flange” frames tend to suffer from “shear lag”; this result in mid-face “flange” columns being less stresses than the corner columns and, therefore, not contributing as fully as they could to the flange action.7Tube-in-Tube or Hull-Core StructuresThis variation of the framed tube consists of an outer framed tube, the “hull” together with an internal elevator and service core (Figure 3-8). The hull and the inner core act jointly in resisting both gravity and lateral loading. In a steel structure the core may consist of braced frames, whereas in a concrete structure it would consist of an assembly of shear walls.Figure 3-8 Tube-in-tubeTo some extent, the outer framed tube and the inner core interact horizontally as the shear and flexural components of a wall-frame structure, with the benefit of increase lateral stiffness. However, the structural tube usually adopts a highly dominant role because of its much greater structural depth.8Braced-Tube StructuresAnother way of improving the efficiency of the framed tube, thereby increasing its potential for greater heights as well as allowing greater spacing between the columns, is to add diagonal bracing to the faces of the tube. This arrangement was first used in a steel structure in 1969, in Chicago’s John Hancock Building (Figure 3-9). Because the diagonal of a braced tube are connected to the columns at each intersection, they virtually eliminate the effects of shear lag in both the flange and web frames. As a result, the structure behaves under lateral loading more like a braced frame, with greatly diminished bending in the members of the frames. Consequently, the spacing of the columns can be larger and the depth of the spandrels less, thereby allowing larger size windows than in the conventional tube structure.Figure 3-9 Braced-Tube StructuresIn the braced-tube structure the bracing contributes also to the improved performance of the tube in carrying gravity loading: differences between gravity load stresses in the columns are evened out by the braces transferring loading from the more highly to the less highly stressed columns.9Bundled-Tube StructuresThis structural form has been used for the Sears Tower in Chicago. The Sears Tower consists of four parallel rigid steel frames in each orthogonal direction, interconnected to form nine “bundled” tubes. As in the single-tube structure, the frames in the direction of lateral loading serves as “webs” of the vertical cantilever, with the normal f rame acting as “flanges”.The introduction of internal webs greatly reduces the shear lag in the flanges; consequently their columns are more evenly stressed than in the single-tube structure, and their contribution to the lateral stiffness is great. This allows columns of the frames to be spaced further apart and to be less obtrusive. In the Sears Tower, advantage was taken of the bundled form to discontinue some of the tubes, and so reduce the plan of the building at stages up to the height.New Words and Expressionsbraced frame 支撑框架braced-tube 桁架筒bundled-tube 束筒coupling beam 连梁coupled shear wall 联肢墙framed tube 框筒inter-storey drift 层间位移propping [ 'prɔpiŋ ] n. 支撑rigid frame 框架shear lag 剪力滞后spandrel [ 'spændrəl ] n. 上下层窗间墙stairway [ 'stεəwei ] n. 楼梯transfer beam 转换粱tube-in-tube / hull-core 筒中筒wall-frame structure 框架-剪力墙结构Exercises1Please name the types of earthquake resistant structural systems.2How does a rigid frame structure resist the gravity load and lateral load?3 Why are shear walls in both directions preferred?4 How are the loads shared between frame and tube in a framed-tube structure?Reading MaterialsPassage OneReinforced Concrete StructuresConcrete and reinforced concrete are used as building materials in every country. In many, including the United States and Canada, reinforced concrete is a dominant structural material in engineered construction. The universal nature of reinforced concrete construction stems from thewide availability of reinforcing bars and the constituents of concrete, gravel, sand, and cement, the relatively simple skills required in concrete construction, and the economy of reinforced concrete compared to other forms of construction. Concrete and reinforced concrete are used in bridges, buildings of all sorts, underground structures, water tanks, television towers, offshore oil exploration and production structures, dams, and even in ships.1Mechanics of Reinforced ConcreteConcrete is strong in compression but weak in tension. As a result, cracks develop whenever loads, or restrained shrinkage or temperature changes, give rise to tensile stresses in excess of the tensile strength of the concrete. In the plain concrete beam, the moments due to applied loads are resisted by an internal tension-compression couple involving tension in the concrete. Such a beam fails very suddenly and completely when the first crack forms. In a reinforced concrete beam, steel bars are embedded in the concrete in such a way that the tension forces needed for moment equilibrium after the concrete cracks can be developed in the bars.The construction of a reinforced concrete member involves building a form or mold in the shape of the member being built. The form must be strong enough to support the weight and hydrostatic pressure of the wet concrete, and any forces applied to it by workers, concrete buggies, wind, and so on. The reinforcement is placed in this form and held in place during the concreting operation. After the concrete has h ardened, the forms are removed.2Factors Affecting Choice of Concrete for a StructureThe choice of whether a structure should be built of concrete, steel, masonry, or timber depends on the availability of materials and on a number of value decisions.(1)EconomyFrequently, the foremost consideration is the overall cost of the structure. This is, of course, a function of the costs of the materials and the labor necessary to erect them. Frequently, however, the overall cost is affected as much or more by the overall construction time since the contractor and owner must allocate money to carry out the construction and will not receive a return on this investment until the building is ready for occupancy. As a result, financial savings due to rapid construction may more than offset increased material costs. Any measures the designer can take to standardize the design and forming will generally pay off in reduced overall costs.In many cases the long-term economy of the structure may be more important than the first cost. As a result, maintenance and durability are important c onsiderations.(2)Suitability of Material for Architectural and Structural F unctionA reinforced concrete system frequently allows the designer to combine the architectural and structural functions. Concrete has the advantage that it is placed in a plastic condition and is given the desired shape and texture by means of the forms and the finishing techniques. This allows such elements as flat plates or other types of slabs to serve as load-bearing elements while providing the finished floor and ceiling surfaces. Similarly, reinforced concrete wails can provide architecturally attractive surfaces in addition to having the ability to resist gravity, wind, or seismic loads. Finally, the choice of size or shape is governed by the designer and not by the availability of standard manufactured members.(3)Fire ResistanceThe structure in a building must withstand the effects of a fire and remain standing while the building is evacuated and the fire is extinguished. A concrete building inherently has a 1- to 3-hour fire rating without special fireproofing or other details. Structural steel or timber buildings must befireproofed to attain similar fire ratings.(4)RigidityThe occupants of a building may be disturbed if their building oscillates in the wind or the floors vibrate as people walk by. Due to the greater stiffness and mass of a concrete structure, vibrations are seldom a problem.(5)Low MaintenanceConcrete members inherently require less maintenance than do structural steel or timber members. This is particularly true if dense, air-entrained concrete has been used for surfaces exposed to the atmosphere, and if care has been taken in the design to provide adequate drainage off and away from the structure.(6)Availability of MaterialsSand, gravel, cement, and concrete mixing facilities are very widely available, and reinforcing steel can be transported to most job sites more easily than can structural steel. As a result, reinforced concrete is frequently used in remote areas.On the other hand, there are a number of factors that may cause one to select a material other than reinforced concrete. These include:(1)Low Tensile StrengthAs stated earlier, the tensile strength of concrete is much lower than its compressive strength (about 1/10), and hence concrete is subject to cracking. In structural uses this is overcome by using reinforcement to carry tensile forces and limit crack widths to within acceptable values. Unless care is taken in design and construction, however, these cracks may be unsightly or may allow penetration of water.(2)Forms and ShoringThe construction of a cast-in-place structure involves three steps not encountered in the construction of steel or timber structures. These are the construction of the forms, the removal of these forms, and propping or shoring the new concrete to support its weight until its strength is adequate. Each of these steps involves labor and/or materials which are not necessary with other forms of construction.(3)Relatively Low Strength per Unit of Weight or VolumeThe compressive strength of concrete is roughly 5% to 10% that of steel, while its unit density is roughly 30% that of steel. As a result, a concrete structure requires a larger volume and a greater weight of material than does a comparable steel structure. As a result, long-span structures are often built from steel.(4)Time-dependent Volume ChangesBoth concrete and steel undergo approximately the same amount of thermal expansion and contraction. Because there is less mass of Steel to be heated or cooled, and because steel is a better conductor than concrete, a steel structure is generally affected by temperature changes to a greater extent than is a concrete structure. On the other hand, concrete undergoes drying shrinkage, which, if restrained, may cause deflections or cracking. Furthermore, deflections will tend to increase with time, possibly doubling, due to creep of the concrete under sustained loads.3Building CodesThe first set of building regulations for reinforced concrete were drafted under the leadership of Professor Morsch of the University of Stuttgart and were issued in Prussia in 1904. Design regulations were issued in Britain, France, Austria, and Switzerland between 1907 and 1909.The American Railway Engineering Association appointed a Committee on Masonry in 1890. In 1903 this committee presented specifications for Portland cement concrete. Between 1908 and 1910 a series of committee reports led to the Standard Building Regulations for the Use of Reinforced Concrete published in 1910 by the National Association of Cement Users which subsequently became the American Concrete Institute.A Joint Committee on Concrete and Reinforced Concrete was established in 1904 by the American Society of Civil Engineers, American Society for Testing and Materials, the American Railway Engineering Association, and the Association of American Portland Cement Manufactures. This group was later joined by the American Concrete Institute. Between 1904 and 1910 the Joint Committee carried out research. A preliminary report issued in 1913 lists the more important papers and books on reinforced concrete published between 1898 and 1911. The final report of this committee was published in 1916. The history of reinforced concrete building codes in the United States was reviewed in 1954 by Kerekes and Reid.The design and construction of buildings is regulated by municipal bylaws called building codes. These exist to protect the public health and safety. Each city and town is free to write or adopt its own building code, and in that city or town, only that particular code has legal status. Because of the complexity of building code writing, cities in the United States generally base their building codes on one of three model codes: the Uniform Building Code, the Standard Building Code, or the Basic Building Code. These codes cover such things as use and occupancy requirements, fire requirements, heating and ventilating requirements, and structural design.The definitive design specification for reinforced concrete buildings in North America is the Building Code Requirements for Reinforced Concrete (ACI-318-95), which is explained in a Commentary.This code, generally referred to as the ACI Code, has been incorporated in most building codes in the United States and serves as the basis for comparable codes in Canada, New Zealand, Australia, and parts of Latin America. The ACI Code has legal status only if adopted in a local building code.Each nation or group of nations in Europe has its own building code for reinforced concrete. The CEB-FIP Model Code for Concrete Structures is intended to serve as the basis for future attempts to unify European codes. This code and the ACI Code are similar in many ways.Passage TwoEarthquake Induced Vibration of Structures1Seismicity and Ground MotionsThe most common cause of earthquakes is thought to be the violent slipping of rock masses along major geological fault lines in the Earth’s crust, or lithosphere. These fault lines divide the global crust into about 12 major tectonic plates, which are rigid, relatively cool slabs about 100km thick. Tectonic plates float on the molten mantle of the Earth and move relative to one another at the rate of 10 to 100mm/year.The basic mechanism causing earthquakes in the plate boundary regions appears to be that the continuing deformation of the crustal structure eventually leads to stresses which exceed the material strength. A rupture will then initiate at some critical point along the fault line and willpropagate rapidly through the highly stressed material at the plate boundary. In some cases, the plate margins are moving away from one another. In those cases, molten rock appears from deep in the Earth to fill the gap, often manifesting itself as volcanoes. If the plates are pushing together, one plate tends to dive under the other and, depending on the density of the material, it may resurface in the form of mountains and valleys. In both these scenarios, there may be volcanoes and earthquakes at the plate boundaries, both being caused by the same mechanism of movement in the Earth's crust. Another possibility is that the plate boundaries will slide sideways past each other, essentially retaining the local surface area of the plate. It is believed that about three quarters of the world's earthquakes are accounted for by this rubbing-striking-slipping mechanism, with ruptures occurring on faults on boundaries between tectonic plates. Earthquake occurrence maps tend to outline the plate boundaries. Such earthquakes are referred to as interplate earthquakes.Earthquakes also occur at locations away from the plate boundaries. Such events are known as intraplate earthquakes and they are much less frequent than interplate earthquakes. Because tectonic plates are not homogeneous or isotropic, areas of local high stress are developed as the plate attempts to move as a rigid body. Accordingly, rupture within the plate, and the consequent release of energy, are believed to give rise to these intraplate events.The po int in the Earth’s crustal system where an earthquake is initiated (the point of rupture) is called the hypocenter or focus of the earthquake. The point on the Earth’s surface directly above the focus is called the epicenter and the depth of the focus is the focal depth. Earthquake occurrence maps usually indicate the location of various epicenters of past earthquakes and these epicenters are located by seismological analysis of the effect of earthquake waves on strategically located receiving instruments called seismometers.When an earthquake occurs, several types of seismic wave are radiated from the rupture. The most important of these are the body waves (primary P and secondary S waves). P waves are essentially sound waves traveling through the Earth, causing particles to move in the direction of wave propagation with alternate expansions and compressions. They tend to travel through the Earth with velocities of up to 8000m/sec (up to 30 times faster than sound waves through air). S waves are shear waves with particle motion transverse to the direction of propagation. S waves tend to travel at about 60% of the velocity of P waves, so they always arrive at seismometers after the P waves. The-time lag between arrivals often provides seismologists with useful information about the distance of the epicenter from the recorder.2Measurement of EarthquakesEarthquakes are complex multi-dimensional phenomena, the scientific analysis of which requires measurement. Prior to the invention of modern scientific instruments, earthquakes were qualitatively measured by intensity, which differed from point-to-point. With the deployment of seismometers, an instrumental quantification of the entire earthquake event-the unique magnitude of the event-became possible.(1)MagnitudeThe total strain energy released during an earthquake is known as the magnitude of the earthquake and it is measured on the Richter scale. It is defined quite simply as the amplitude of the recorded vibrations on a particular kind of seismometer located at a particular distance from the epicenterM L = log A –log A o (3-1)。
土木工程外文翻译-原文

外文原文Response of a reinforced concrete infilled-frame structure to removal of twoadjacent columnsMehrdad Sasan_iNortheastern University, 400 Snell Engineering Center, Boston, MA 02115, UnitedStatesReceived 27 June 2007; received in revised form 26 December 2007; accepted 24January 2008Available online 19 March 2008AbstractThe response of Hotel San Diego, a six-story reinforced concrete infilled-frame structure, is evaluated following the simultaneous removal of two adjacent exterior columns. Analytical models of the structure using the Finite Element Method as well as the Applied Element Method are used to calculate global and local deformations. The analytical results show good agreement with experimental data. The structure resisted progressive collapse with a measured maximum vertical displacement of only one quarter of an inch mm). Deformation propagation over the height of the structure and the dynamic load redistribution following the column removal are experimentally and analytically evaluated and described. The difference between axial and flexural wave propagations is discussed. Three-dimensional Vierendeel (frame) action of the transverse and longitudinal frames with the participation of infill walls is identified as the major mechanism for redistribution of loads in the structure. The effects of two potential brittle modes of failure (fracture of beamsections without tensile reinforcement and reinforcing bar pull out) are described. The response of the structure due to additional gravity loads and in the absence of infill walls is analytically evaluated.c 2008 Elsevier Ltd. All rights reserved.Keywords: Progressive collapse; Load redistribution; Load resistance; Dynamic response; Nonlinear analysis; Brittle failure 1. IntroductionThe principal scope of specifications is to provide general principles and computational methods in order to verify safet y of structures. The “ safety factor ” , which according t o modern trends is independent of the nature and combination of the materials used, can usually be defined as the rati o between the conditions. This ratio is also proportional tothe inverse of the probability ( risk ) of failure of the structure. Failure has tobe considerednot only asoverall collapseo f thestructure but also as unserviceability or,accordingtes of limit stateof the structure; failure of somesections and subsequent transformation of the structure into a mechanism; failureby fatigue;elastic or plasticdeformation or creep that cause a substantial change of the geometry of the structure; and sensitivity of the structure to alte rnating loads, to fire and to explosions.o a more precise.Common definition. As the reaching of alimit state ” which causes the construction not to acco mplish the task itwas designed for. There aretwo categori(1)Ultimate limit sate, which corresponds to the highest valu e of the load-bearingcapacity. Examples includelocal buckling or global instability(2)Service limit states, which are functions of the use and durability of the structure.Examples include excessive deformations and displacements without instability; early or exces sive cracks; large vibrations; and corrosion.Computational methods used to verify structures with respectto the different safety conditions can be separated into:(1)Deterministic methods, in which the main parameters are co nsidered as nonrandom parameters.(2)Probabilistic methods, in which the main parameters are co nsidered as random parameters.Alternatively, with respect to the different use of factorsof safety, computational methods can be separated into:(1)Allowable stress method, in which the stresses computed un der maximum loads are compared with the strength of the mat erial reduced by given safety factors.(2)Limit states method, in which the structure may be proportioned on the basis of its maximum strength . This strength,as determined by rational analysis, shall not be less thanthat required to support a factored load equal to the sumof the factored live load and dead load ( ultimate state).The stresses corresponding to working ( service ) conditionswith unfactored live and dead loads are compared with pres cribed values ( service limit state ) . From the four possible combinations of the first two and second two methods,we can obtain some useful computational methods. Generally, t wo combinations prevail:(1)deterministic methods, which make use of allowable stresses. (2)Probabilistic methods, which make use of limit states.The main advantage of probabilistic approaches is that, at l east in theory, it is possible to scientifically take into account all random factors of safety, which are then combine d to define the safety factor. probabilistic approaches depend upon :(1) Random distribution of strength of materials with respectto the conditions of fabrication and erection ( scatter of the values of mechanical properties through out the structure ); (2) Uncertainty of the geometry of the cross-section sand of the structure( faults and imperfections due to fab rication and erection of the structure );(3) Uncertainty of the predicted live loads and dead loadsacting on the structure; (4)Uncertainty related to the approx imation of the computational method used ( deviation of theactual stresses from computed stresses ). Furthermore, proba bilistic theories mean t hat the allowable risk can be basedon several factors, such as :(1) Importance of the construction and gravity of the damageby its failure; (2)Number of human lives which can be thr eatened by this failure; (3)Possibility and/or likelihood of repairing the structure; (4) Predicted life of the structure.All these factors are related to economic and social consi derations such as:(1) Initial cost of the construction;(2) Amortization funds for the duration of the construction;Adverse impact on society; Moral and psychological views.part of mitigation programs to reduce the likelihood of mass casualties following local damagein structures, the General Services Administration [ 1] and the Department of Defense [ 2] developed regulations to evaluateprogressive collapse resistance of structures. ASCE/SEI 7 [ progressive collapse as the spread of an initial local failure fromelement to element eventually resulting in collapse of an entire structure or a disproportionately large part of it. Following the approaches proposed by Ellinwood and Leyendecker [4], ASCE/SEI 7 [ 3] defines twogeneral methods for structural design of buildings to mitigate damage due tothe difficulty of carrying out a complete probabilistic analysishas tobe taken intoaccount. For such an analysis t he laws of the distribution of the live load and its induc ed stresses, of the scatter of mechanicalproperties ofmaterials, and ofthe geometryof the cross-sections and the st ructure have to be known.Furthermore, itis difficult to i nterpret the interaction between the law of distribution of strength and that of stresses because both depend uponthe natureof thematerial, onthe cross-sectionsand upon theload acting on the structure. These practicaldifficulties caconstruction ety at differentapplyn be overcomethe optimum cost. However, is toin two ways. The first factor, allows(3)Cost of physical and materialdamage due to the failureof the construction;(4) (5)The definition of all these parameters, for a given safsafety factorsto the material andto the loads, without necessarily adopting the probabilistic criterion.The second is an approximate probabilisticmethod which introducessome simplifying assumptions ( semi-probabilistic methods ) As3] definesprogressive collapse: indirect and direct design methods. General building codes and standards [ 3,5] use indirect design by increasingoverall integrity of structures. Indirect design is also used in DOD [ 2]. Although the indirect design method can reduce the risk of progressive collapse [ 6,7] estimation of post-failure performance ofstructures designed based on such a method is not readily possible. One approach based on direct design methods to evaluate progressive collapse of structures is to study the effects of instantaneous removal of load-bearing elements, such as columns. GSA [ 1] and DOD [ 2] regulations require removal of one load bearing element. These regulations are meant to evaluate general integrity of structures and their capacity of redistributing the loads following severe damage to only one element. While such an approach provides insight as to the extent to which the structures are susceptible to progressive collapse, in reality, the initial damagecan affect more than just one column. In this study, using analytical results that are verified against experimental data, the progressive collapse resistance of the Hotel San Diego is evaluated, following the simultaneous explosion (sudden removal) of two adjacent columns, one of which was a corner column. In order to explode the columns, explosives were inserted into predrilled holes in the columns. The columns were then well wrapped with a few layers of protective materials. Therefore, neither air blast nor flying fragments affected the structure.I. A sGuih view of hcxel San IJiego, Center sinicmrr is siudrd in ihis paper.Lig.工S<cund 门血卜「of building (Looking south>2. Buildi ng characteristicsHotel San Diego was con structed in 1914 with a south annex added in1924. The annex in cluded two separate build in gs. Fig. 1 shows a south view of the hotel. Note that in the picture, the first and third stories of the hotel are covered with black fabric. The six story hotel had a non-ductile rein forced con crete (RC) frame structure with hollow clay tile exterior infill walls. The in fills in the annex con sisted of two withes (layers) of clay tiles with a total thick ness of about 8 in (203 mm). The height of the first floor was about 190 - 800 m). The heightof other floors and that of the top floor were 100 - 600 m) and 160 - 1000 m), respectively. Fig. 2 shows the sec ond floor of one of the annexbuild in gs. Fig. 3 shows a typical pla n of this build ing, whose resp onsefollowing the simultaneous removal (explosion) of columns A2 and A3 in the first (ground) floor is evaluated in this paper. The floor system consisted of one-way joists running in the longitudinal direction (North - South), as shown in Fig. 3 . Based oncompression tests of two concrete samples, the average concrete compressive strength was estimated at about 4500 psi (31 MPa) for a standard concrete cylinder. The modulus of elasticity of concrete was estimated at 3820 ksi (26 300 MPa) [ 5]. Also, based on tension tests of two steel samples having 1/2 in mm) square sections, the yield and ultimate tensile strengths were found to be 62 ksi (427 MPa) and 87 ksi (600 MPa), respectively. The steel ultimate tensile strain was measured at . The modulus of elasticity of steel was set equal to 29 000 ksi (200 000 MPa). The building was scheduled to be demolished by implosion. As part of the demolition process, the infill walls were removed from the first and third floors. There was no live load in the building. All nonstructural elements including partitions, plumbing, and furniture were removed prior to implosion.Only beams, columns, joist floor and infill walls on the peripheral beams were present.3. SensorsConcrete and steel strain gages were used to measure changes in strains of beams and columns. Linear potentiometers were used to measure global and local deformations. The concrete strain gages were in (90 mm) long having a maximumstrain limit of ±. The steel strain gages could measure up to a strain of ±. The strain gages could operate up to a several hundred kHz sampling rate. The sampling rate used in the experiment was 1000 Hz. Potentiometers were used to capture rotation (integral of curvature over a length) of the beamend regions and global displacementin the building, as described later. The potentiometers had a resolution of about in mm) and a maximumoperational speed of about 40 in/s m/s), while the maximum recorded speed in the experime nt was about 14 in/sm/s).B丄6E4.98 m4 gm m4gtp34brE3P® 3. TS'pical plan of Hokl San (South Anne?! I First floor K mowdl columns arc crossed.Rg.丄 Li:ifui\.^J i K?nc dk uil u>fculuiiin^ JXH J 4a) Beiu 叮 A3^B3 LII stvuiki fluui; uiid (bi Bcdiii M- A 24. Fin ite eleme nt modelUsing the finite element method (FEM), a model of the building was developed in the SAP2000 [8] computer program. The beams and colu mnsare modeled with Berno ulli beam eleme nts. Beams have T or L sect ions with effective flange width on each side of the web equal to four times the slab thick ness [5]. Plastic hin ges are assig ned to all possiblelocations where steel bar yielding can occur, including the ends of eleme nts as well as the reinforcing bar cut-off and bend locati ons. The characteristics of the plastic hinges are obtained using section analyses of the beams and columns and assuminga plastic hinge length equal to half of the section depth. The current version of14-41 —■」5 0 (18 rmn)VE«TTiXflrtl<EF)直由 t&A IFffl FA1l -20 SflHTGnn^Msffir-3i 口 W (10 EK IIM 吟IA2J 口軸]-iftlwMl5CIB ITE2 □ SiS" 1A mm).#2 ^py ^ur wren [M rnm V' gT FPH JSAP2000 [ 8] is not able to track formation of cracks in the elements. In order to find the proper flexural stiffness of sections, an iterative procedure is used as follows. First, the building is analyzed assuming all elements are uncracked. Then, moment demands in the elements are compared with their cracking bending moments, Mcr . The moment of inertia of beam and slab segments are reduced by a coefficient of [ 5], where the demand exceeds the Mcr. The exterior beam cracking bending moments under negative and positive moments, are 516 k in kN m) and 336 k in kN m), respectively. Note that no cracks were formed in the columns. Then the building is reanalyzed and moment diagrams are re-evaluated. This procedure is repeated until all of the cracked regions are properly identified and modeled.The beams in the building did not have top reinforcing bars except at the end regions (see Fig. 4 ). For instance, no top reinforcement was provided beyond the bend in beam A1 - A2, 12 inches away from the face of column A1 (see Figs. 4 and 5). To model the potential loss of flexural strength in those sections, localized crack hinges were assigned at the critical locations where no top rebar was present. Flexural strengths of the hinges were set equal to Mcr. Such sections were assumed to lose their flexural strength when the imposed bending moments reached Mcr.The floor system con sisted of joists in the Ion gitudi nal directi on(North — South). Fig. 6 shows the cross sect ion of a typical floor. I norder to acco unt for pote ntial non li near resp onse of slabs and joists, floors are molded by beam eleme nts. Joists are modeled with T-secti ons, having effective flange width on each side of the web equal to four times the slab thick ness [ 5]. Give n the large joist spac ing betwee n axes 2and 3, two rectangular beamelements with 20-inch wide sections are usedbetwee n the joist and the Ion gitud inal beams of axes 2 and 3 to model the slab in the Ion gitud inal directi on. To model the behavior of the slab in the transverse direction, equally spaced parallel beams with20-i nch wide recta ngular sect ions are used. There is a differe nee betwee n the shear flow in the slab and that in the beamelements with rectangular secti ons modeli ng the slab. Because of this, the torsi onal stiff ness is setequal to on e-half of that of the gross sect ions [The building had infill walls on 2nd, 4th, 5th and 6thfloors on the spandrel beams with some openings . windows and doors). As9].Fig, 5, Location of bends in beam top re infnicenient (in an adjacent inncKbuilding at u location similar to beam Al A2. close to column A11mentioned before and as part of the demolition procedure, the infill walls in the 1st and 3rd floors were removed before the test. The infill walls were made of hollow clay tiles, which were in good condition. The net area of the clay tiles was about 1/2 of the gross area. The in-plane action of the infill walls contributes to the building stiffness and strength and affects the building response. Ignoring the effects of the infill walls and excluding them in the model would result in underestimating the building stiffness and strength.Using the SAP2000 computer program [8], two types of modeling for the infills are considered in this study: one uses two dimensional shell elements (Model A) and the other uses compressive struts (Model B) as suggested in FEMA356 [10] guidelines. . Model A (infills modeled by shell elements)Infill walls are modeled with shell elements. However, the current version of the SAP2000 computer program includes only linear shell elements and cannot account for cracking. The tensile strength of the infill walls is set equal to 26 psi, with a modulus of elasticity of 644 ksi [10]. Because the formation ofcracks has a significant effect on the stiffness of the infill walls, the following iterative procedure is used to account for crack formation:(1) Assuming the infill walls are linear and uncracked, a nonlinear time history analysis is run. Note that plastic hinges exist in the beam elements and the segments of the beamelements where momentdemandexceeds the cracking moment have a reduced moment of inertia.(2) The cracking pattern in the infill wall is determined by comparingstresses in the shells developed during the analysis with the tensile strength of infills.(3) Nodes are separated at the locations where tensile stress exceeds tensile strength. These steps are continued until the crack regions are properly modeled.. Model B (infills modeled by struts)Infill walls are replaced with compressive struts as described in FEMA 356 [ 10] guidelines. Orientations of the struts are determined from the deformed shape of the structure after column removal and the location of openings.. Column removalRemoval of the columns is simulated with the following procedure.(1) The structure is analyzed under the permanent loads and the internal forces are determined at the ends of the columns, which will be removed.(2) The model is modified by removing columns A2 and A3 on the first floor. Again the structure is statically analyzed under permanent loads. In this case, the internal forces at the ends of removed columns found in the first step are applied externally to the structure along with permanent loads. Note that the results of this analysis are identical to those of step 1.(3) The equal and opposite column end forces that were applied in the second step are dynamically imposed on the ends of the removed column within one millisecond [ 11] to simulate the removal of the columns, anddynamic analysis is conducted.. Comparison of analytical and experimental resultsThe maximum calculated vertical displacement of the building occursFig. 7 shows the experimental and analytical (Model A) at joint A3 in the second floor.vertical displacements of this joint (the AEMresults will be discussed in the next section). Experimental data is obtained using therecordings of three potentiometers attached to joint A3 on one of their ends,and to the ground on the other ends. The peak displacements obtainedexperimentally and analytically (Model A) are in mm) and in mm),respectively, which differ only by about 4%. The experimental and analyticaltimes corresponding to peak displacement are s and s, respectively. Theanalytical results show a permanent displacement of about in mm), which isabout 14% smaller than the corresponding experimental value of in mm).Fig. 8 compares vertical displacement histories of joint A3 in the second floor estimated analytically based on Models A and B. As can be seen, modeling infills with struts (Model B) results in a maximumvertical displacement of joint A3 equal to about in mm), which is approximately 80% larger than the value obtained from Model A. Note that the results obtained from Model A are in close agreement with experimental results (see Fig. 7 ), while Model B significantly overestimates the deformation of the structure. If the maximum vertical displacement were larger, the infill walls were more severely cracked and the struts were more completely formed, the difference between the results of the two models (Models A and B) would be smaller.Fig. 9 compares the experimental and analytical (Model A) displacement of joint A2 in the second floor. Again, while the first peak vertical displacement obtained experimentally and analytically are in good agreement, the analytical permanent displacement under estimates the experimental value.Analytically estimated deformed shapes of the structure at the maximumvertical displaceme nt based on Model A are show n inFig. 10 with a magn ificati on factor of 200. The experime ntally measured deformed shape over the end regi ons of beams A1 —A2 and A3 —B3 in the sec ond floor are represented in the figure by solid lines. A total of 14 potentiometers were located at the top and bottom of the end regions of the second floor beams A1— A2 and A3 —B3, which were the most critical elements in load recordings were used to calculate rotation between the sections where the potentiometer ends were connected. This was done by first finding the difference between the recorded deformations at the top and bottom of the beam, and then dividing the value by the distance (along the height of the beamsection) between the two potentiometers. The expected deformed shapes between the measured end regions of the second floor beams are shown by dashed lines. As can be seen in the figures, analytically estimated deformed shapes of the beams are in good agreement with experimentally obtained deformed shapes.redistributio n. The beam top and corresponding bottom potentiometer3Ktg. in hf Kid jotsl tkwr 疔!-0-3 I 1 1 1 1 1 1 1l 1 1 1 1 1 1 1O J O Q.2 04 QiG 08 10 12 14 1.6Tirre (s)7- Lix peri mental end analytical vertical displace m^nts cf joinl A3 in second flocH■_0.0-柑」■gr7^ -riovBA L 口--2 500 02 C.4 D6 0B 1.D 12 1 J 1.CTmaFi|, S, Vcrtital Jbfplaxmcm hiisiorics wf joint A3 in flLMn c^tiiTiatcdaruljtit^II Y U IM U on M^xkls A airnd B fJTM ),Fi^. IQ Ajk41)ik J 'H;M. Midte 】/U dkRAiisJ Miiipcs yf HribLtiiiv iScumd flwi rxpt litHaulh* tMiitukd Urruiaikcd 如厅产、alt 虐】沁 寸凯曲対). Analytical results of Model A show that only two plastic hinges areformed in dicati ng rebar yield ing. Also, four sect ions that did not haven egative (top) rein forceme nt, reached crack ing mome nt capacities andtherefore cracked. Fig. 10 shows the locations of all the formed plastic hin ges and cracks.B3F :it .比 bp. \ IJIL 'akil JI d .ILJ I ^I'.iLd ;山spldf I ;L II ul' UHEIL A 2 ia id do >L【uo -Lv一匸严.>A1 a noo hP Floor* HVHMC 叭 PCME■ 0«d4fe AeihClMiiOHti 册Et 列。
【建筑工程管理】土木工程英语文献原文及中文翻译

Civil engineering introduction papers[英语原文]Abstract: the civil engineering is a huge discipline, but the main one is building, building whether in China or abroad, has a long history, long-term development process. The world is changing every day, but the building also along with the progress of science and development. Mechanics findings, material of update, ever more scientific technology into the building. But before a room with a tile to cover the top of the house, now for comfort, different ideas, different scientific, promoted the development of civil engineering, making it more perfect.[key words] : civil engineering; Architecture; Mechanics, Materials.Civil engineering is build various projects collectively. It was meant to be and "military project" corresponding. In English the history of Civil Engineering, mechanical Engineering, electrical Engineering, chemical Engineering belong to to Engineering, because they all have MinYongXing. Later, as the project development of science and technology, mechanical, electrical, chemical has gradually formed independent scientific, to Engineering became Civil Engineering of specialized nouns. So far, in English, to Engineering include water conservancy project, port Engineering, While in our country, water conservancy projects and port projects also become very close and civilengineering relatively independent branch. Civil engineering construction of object, both refers to that built on the ground, underground water engineering facilities, also refers to applied materials equipment and conduct of the investigation, design and construction, maintenance, repair and other professional technology.Civil engineering is a kind of with people's food, clothing, shelter and transportation has close relation of the project. Among them with "live" relationship is directly. Because, to solve the "live" problem must build various types of buildings. To solve the "line, food and clothes" problem both direct side, but also a indirect side. "Line", must build railways, roads, Bridges, "Feed", must be well drilling water, water conservancy, farm irrigation, drainage water supply for the city, that is direct relation. Indirectly relationship is no matter what you do, manufacturing cars, ships, or spinning and weaving, clothing, or even production steel, launch satellites, conducting scientific research activities are inseparable from build various buildings, structures and build all kinds of project facilities.Civil engineering with the progress of human society and development, yet has evolved into large-scale comprehensive discipline, it has out many branch, such as: architectural engineering, the railway engineering, road engineering, bridge engineering, special engineeringstructure, water and wastewater engineering, port engineering, hydraulic engineering, environment engineering disciplines. [1]Civil engineering as an important basic disciplines, and has its important attributes of: integrated, sociality, practicality, unity. Civil engineering for the development of national economy and the improvement of people's life provides an important material and technical basis, for many industrial invigoration played a role in promoting, engineering construction is the formation of a fixed asset basic production process, therefore, construction and real estate become in many countries and regions, economic powerhouses.Construction project is housing planning, survey, design, construction of the floorboard. Purpose is for human life and production provide places.Houses will be like a man, it's like a man's life planning environment is responsible by the planners, Its layout and artistic processing, corresponding to the body shape looks and temperament, is responsible by the architect, Its structure is like a person's bones and life expectancy, the structural engineer is responsible, Its water, heating ventilation and electrical facilities such as the human organ and the nerve, is by the equipment engineer is responsible for. Also like nature intact shaped like people, in the city I district planning based on build houses, and is the construction unit, reconnaissance unit, design unit of various designengineers and construction units comprehensive coordination and cooperation process.After all, but is structural stress body reaction force and the internal stress and how external force balance. Building to tackle, also must solve the problem is mechanical problems. We have to solve the problem of discipline called architectural mechanics. Architectural mechanics have can be divided into: statics, material mechanics and structural mechanics three mechanical system. Architectural mechanics is discussion and research building structure and component in load and other factors affecting the working condition of, also is the building of intensity, stiffness and stability. In load, bear load and load of structure and component can cause the surrounding objects in their function, and the object itself by the load effect and deformation, and there is the possibility of damage, but the structure itself has certain resistance to deformation and destruction of competence, and the bearing capacity of the structure size is and component of materials, cross section, and the structural properties of geometry size, working conditions and structure circumstance relevant. While these relationships can be improved by mechanics formula solved through calculation.Building materials in building and has a pivotal role. Building material is with human society productivity and science and technologyimproves gradually developed. In ancient times, the human lives, the line USES is the rocks andTrees. The 4th century BC, 12 ~ has created a tile and brick, humans are only useful synthetic materials made of housing. The 17th century had cast iron and ShouTie later, until the eighteenth century had Portland cement, just make later reinforced concrete engineering get vigorous development. Now all sorts of high-strength structural materials, new decoration materials and waterproof material development, criterion and 20th century since mid organic polymer materials in civil engineering are closely related to the widely application. In all materials, the most main and most popular is steel, concrete, lumber, masonry. In recent years, by using two kinds of material advantage, will make them together, the combination of structure was developed. Now, architecture, engineering quality fit and unfit quality usually adopted materials quality, performance and using reasonable or not have direct connection, in meet the same technical indicators and quality requirements, under the precondition of choice of different material is different, use method of engineering cost has direct impact.In construction process, building construction is and architectural mechanics, building materials also important links. Construction is to the mind of the designer, intention and idea into realistic process, from the ancient hole JuChao place to now skyscrapers, from rural to urbancountry road elevated road all need through "construction" means. A construction project, including many jobs such as dredging engineering, deep foundation pit bracing engineering, foundation engineering, reinforced concrete structure engineering, structural lifting project, waterproofing, decorate projects, each type of project has its own rules, all need according to different construction object and construction environment conditions using relevant construction technology, in work-site.whenever while, need and the relevant hydropower and other equipment composition of a whole, each project between reasonable organizing and coordination, better play investment benefit. Civil engineering construction in the benefit, while also issued by the state in strict accordance with the relevant construction technology standard, thus further enhance China's construction level to ensure construction quality, reduce the cost for the project.Any building built on the surface of the earth all strata, building weight eventually to stratum, have to bear. Formation Support building the rocks were referred to as foundation, and the buildings on the ground and under the upper structure of self-respect and liable to load transfer to the foundation of components or component called foundation. Foundation, and the foundation and the superstructure is a building of three inseparable part. According to the function is different, but in load, under the action of them are related to each other, is theinteraction of the whole. Foundation can be divided into natural foundation and artificial foundation, basic according to the buried depth is divided into deep foundation and shallow foundation. , foundation and foundation is the guarantee of the quality of the buildings and normal use close button, where buildings foundation in building under loads of both must maintain overall stability and if the settlement of foundation produce in building scope permitted inside, and foundation itself should have sufficient strength, stiffness and durability, also consider repair methods and the necessary foundation soil retaining retaining water and relevant measures. [3]As people living standard rise ceaselessly, the people to their place of building space has become not only from the number, and put forward higher requirement from quality are put car higher demands that the environment is beautiful, have certain comfort. This needs to decorate a building to be necessary. If architecture major engineering constitutes the skeleton of the building, then after adornment building has become the flesh-and-blood organism, final with rich, perfect appearance in people's in front, the best architecture should fully embody all sorts of adornment material related properties, with existing construction technology, the most effective gimmick, to achieve conception must express effect. Building outfit fix to consider the architectural space use requirement, protect the subject institutions fromdamage, give a person with beautifulenjoying, satisfy the requirements of fire evacuation, decorative materials and scheme of rationality, construction technology and economic feasibility, etc. Housing construction development and at the same time, like housing construction as affecting people life of roads, Bridges, tunnels has made great progress.In general civil engineering is one of the oldest subjects, it has made great achievements, the future of the civil engineering will occupy in people's life more important position. The environment worsening population increase, people to fight for survival, to strive for a more comfortable living environment, and will pay more attention to civil engineering. In the near future, some major projects extimated to build, insert roller skyscrapers, across the oceanBridges, more convenient traffic would not dream. The development of science and technology, and the earth is deteriorating environment will be prompted civil engineering to aerospace and Marine development, provide mankind broader space of living. In recent years, engineering materials mainly is reinforced concrete, lumber and brick materials, in the future, the traditional materials will be improved, more suitable for some new building materials market, especially the chemistry materials will promote the construction of towards a higher point. Meanwhile, design method of precision, design work ofautomation, information and intelligent technology of introducing, will be people have a more comfortable living environment. The word, and the development of the theory and new materials, the emergence of the application of computer, high-tech introduction to wait to will make civil engineering have a new leap.This is a door needs calm and a great deal of patience and attentive professional. Because hundreds of thousands, even hundreds of thousands of lines to building each place structure clearly reflected. Without a gentle state of mind, do what thing just floating on the surface, to any a building structure, to be engaged in business and could not have had a clear, accurate and profound understanding of, the nature is no good. In this business, probably not burn the midnight oil of courage, not to reach the goal of spirit not to give up, will only be companies eliminated.This is a responsible and caring industry. Should have a single responsible heart - I one's life in my hand, thousands of life in my hand. Since the civil, should choose dependably shoulder the responsibility.Finally, this is a constant pursuit of perfect industry. Pyramid, spectacular now: The Great Wall, the majestic... But if no generations of the pursuit of today, we may also use the sort of the oldest way to build this same architecture. Design a building structure is numerous, but this is all experienced centuries of clarification, through continuousaccumulation, keep improving, innovation obtained. And such pursuit, not confined in the past. Just think, if the design of a building can be like calculation one plus one equals two as simple and easy to grasp, that was not for what? Therefore, a civil engineer is in constant of in formation. One of the most simple structure, the least cost, the biggest function. Choose civil, choosing a steadfast diligence, innovation, pursuit of perfect path.Reference:[1] LuoFuWu editor. Civil engineering (professional). Introduction to wuhan. Wuhan university of technology press. 2007[2] WangFuChuan, palace rice expensive editor. Construction engineering materials. Beijing. Science and technology literature press. 2002[3] jiang see whales, zhiming editor. Civil engineering introduction of higher education press. Beijing.. 1992土木工程概论[译文]摘要:土木工程是个庞大的学科,但最主要的是建筑,建筑无论是在中国还是在国外,都有着悠久的历史,长期的发展历程。
(完整版)土木工程毕业设计外文文献翻译

外文文献翻译Reinforced ConcreteConcrete and reinforced concrete are used as building materials in every country. In many, including the United States and Canada, reinforced concrete is a dominant structural material in engineered construction. The universal nature of reinforced concrete construction stems from the wide availability of reinforcing bars and the constituents of concrete, gravel, sand, and cement, the relatively simple skills required in concrete construction, and the economy of reinforced concrete compared to other forms of construction. Concrete and reinforced concrete are used in bridges, buildings of all sorts underground structures, water tanks, television towers, offshore oil exploration and production structures, dams, and even in ships.Reinforced concrete structures may be cast-in-place concrete, constructed in their final location, or they may be precast concrete produced in a factory and erected at the construction site. Concrete structures may be severe and functional in design, or the shape and layout and be whimsical and artistic. Few other building materials off the architect and engineer such versatility and scope.Concrete is strong in compression but weak in tension. As a result, cracks develop whenever loads, or restrained shrinkage of temperature changes, give rise to tensile stresses in excess of the tensile strength of the concrete. In a plain concrete beam, the moments about the neutral axis due to applied loads are resisted by an internal tension-compression couple involving tension in the concrete. Such a beam fails very suddenly and completely when the first crack forms. In a reinforced concrete beam, steel bars are embedded in the concrete in such a way that the tension forces needed for moment equilibrium after the concrete cracks can be developed in the bars.The construction of a reinforced concrete member involves building a from of mold in the shape of the member being built. The form must be strong enough to support both the weight and hydrostatic pressure of the wet concrete, and any forces applied to it by workers, concrete buggies, wind, and so on. The reinforcement is placed in this form and held in placeduring the concreting operation. After the concrete has hardened, the forms are removed. As the forms are removed, props of shores are installed to support the weight of the concrete until it has reached sufficient strength to support the loads by itself.The designer must proportion a concrete member for adequate strength to resist the loads and adequate stiffness to prevent excessive deflections. In beam must be proportioned so that it can be constructed. For example, the reinforcement must be detailed so that it can be assembled in the field, and since the concrete is placed in the form after the reinforcement is in place, the concrete must be able to flow around, between, and past the reinforcement to fill all parts of the form completely.The choice of whether a structure should be built of concrete, steel, masonry, or timber depends on the availability of materials and on a number of value decisions. The choice of structural system is made by the architect of engineer early in the design, based on the following considerations:1. Economy. Frequently, the foremost consideration is the overall const of the structure. This is, of course, a function of the costs of the materials and the labor necessary to erect them. Frequently, however, the overall cost is affected as much or more by the overall construction time since the contractor and owner must borrow or otherwise allocate money to carry out the construction and will not receive a return on this investment until the building is ready for occupancy. In a typical large apartment of commercial project, the cost of construction financing will be a significant fraction of the total cost. As a result, financial savings due to rapid construction may more than offset increased material costs. For this reason, any measures the designer can take to standardize the design and forming will generally pay off in reduced overall costs.In many cases the long-term economy of the structure may be more important than the first cost. As a result, maintenance and durability are important consideration.2. Suitability of material for architectural and structural function.A reinforced concrete system frequently allows the designer to combine the architectural and structural functions. Concrete has the advantage that it is placed in a plastic condition and is given the desired shapeand texture by means of the forms and the finishing techniques. This allows such elements ad flat plates or other types of slabs to serve as load-bearing elements while providing the finished floor and / or ceiling surfaces. Similarly, reinforced concrete walls can provide architecturally attractive surfaces in addition to having the ability to resist gravity, wind, or seismic loads. Finally, the choice of size of shape is governed by the designer and not by the availability of standard manufactured members.3. Fire resistance. The structure in a building must withstand the effects of a fire and remain standing while the building is evacuated and the fire is extinguished. A concrete building inherently has a 1- to 3-hour fire rating without special fireproofing or other details. Structural steel or timber buildings must be fireproofed to attain similar fire ratings.4. Low maintenance.Concrete members inherently require less maintenance than do structural steel or timber members. This is particularly true if dense, air-entrained concrete has been used for surfaces exposed to the atmosphere, and if care has been taken in the design to provide adequate drainage off and away from the structure. Special precautions must be taken for concrete exposed to salts such as deicing chemicals.5. Availability of materials. Sand, gravel, cement, and concrete mixing facilities are very widely available, and reinforcing steel can be transported to most job sites more easily than can structural steel. As a result, reinforced concrete is frequently used in remote areas.On the other hand, there are a number of factors that may cause one to select a material other than reinforced concrete. These include:1. Low tensile strength.The tensile strength concrete is much lower than its compressive strength ( about 1/10 ), and hence concrete is subject to cracking. In structural uses this is overcome by using reinforcement to carry tensile forces and limit crack widths to within acceptable values. Unless care is taken in design and construction, however, these cracks may be unsightly or may allow penetration of water. When this occurs, water or chemicals such as road deicing salts may cause deterioration or staining of the concrete. Special design details are required in such cases. In the case of water-retaining structures, special details and /of prestressing are required to prevent leakage.2. Forms and shoring. The construction of a cast-in-place structure involves three steps not encountered in the construction of steel or timber structures. These are ( a ) the construction of the forms, ( b ) the removal of these forms, and (c) propping or shoring the new concrete to support its weight until its strength is adequate. Each of these steps involves labor and / or materials, which are not necessary with other forms of construction.3. Relatively low strength per unit of weight for volume.The compressive strength of concrete is roughly 5 to 10% that of steel, while its unit density is roughly 30% that of steel. As a result, a concrete structure requires a larger volume and a greater weight of material than does a comparable steel structure. As a result, long-span structures are often built from steel.4. Time-dependent volume changes. Both concrete and steel undergo-approximately the same amount of thermal expansion and contraction. Because there is less mass of steel to be heated or cooled, and because steel is a better concrete, a steel structure is generally affected by temperature changes to a greater extent than is a concrete structure. On the other hand, concrete undergoes frying shrinkage, which, if restrained, may cause deflections or cracking. Furthermore, deflections will tend to increase with time, possibly doubling, due to creep of the concrete under sustained loads.In almost every branch of civil engineering and architecture extensive use is made of reinforced concrete for structures and foundations. Engineers and architects requires basic knowledge of reinforced concrete design throughout their professional careers. Much of this text is directly concerned with the behavior and proportioning of components that make up typical reinforced concrete structures-beams, columns, and slabs. Once the behavior of these individual elements is understood, the designer will have the background to analyze and design a wide range of complex structures, such as foundations, buildings, and bridges, composed of these elements.Since reinforced concrete is a no homogeneous material that creeps, shrinks, and cracks, its stresses cannot be accurately predicted by the traditional equations derived in a course in strength of materials forhomogeneous elastic materials. Much of reinforced concrete design in therefore empirical, i.e., design equations and design methods are based on experimental and time-proved results instead of being derived exclusively from theoretical formulations.A thorough understanding of the behavior of reinforced concrete will allow the designer to convert an otherwise brittle material into tough ductile structural elements and thereby take advantage of concrete’s desirable characteristics, its high compressive strength, its fire resistance, and its durability.Concrete, a stone like material, is made by mixing cement, water, fine aggregate ( often sand ), coarse aggregate, and frequently other additives ( that modify properties ) into a workable mixture. In its unhardened or plastic state, concrete can be placed in forms to produce a large variety of structural elements. Although the hardened concrete by itself, i.e., without any reinforcement, is strong in compression, it lacks tensile strength and therefore cracks easily. Because unreinforced concrete is brittle, it cannot undergo large deformations under load and fails suddenly-without warning. The addition fo steel reinforcement to the concrete reduces the negative effects of its two principal inherent weaknesses, its susceptibility to cracking and its brittleness. When the reinforcement is strongly bonded to the concrete, a strong, stiff, and ductile construction material is produced. This material, called reinforced concrete, is used extensively to construct foundations, structural frames, storage takes, shell roofs, highways, walls, dams, canals, and innumerable other structures and building products. Two other characteristics of concrete that are present even when concrete is reinforced are shrinkage and creep, but the negative effects of these properties can be mitigated by careful design.A code is a set technical specifications and standards that control important details of design and construction. The purpose of codes it produce structures so that the public will be protected from poor of inadequate and construction.Two types f coeds exist. One type, called a structural code, is originated and controlled by specialists who are concerned with the proper use of a specific material or who are involved with the safe design of a particular class of structures.The second type of code, called a building code, is established to cover construction in a given region, often a city or a state. The objective of a building code is also to protect the public by accounting for the influence of the local environmental conditions on construction. For example, local authorities may specify additional provisions to account for such regional conditions as earthquake, heavy snow, or tornados. National structural codes genrally are incorporated into local building codes.The American Concrete Institute ( ACI ) Building Code covering the design of reinforced concrete buildings. It contains provisions covering all aspects of reinforced concrete manufacture, design, and construction. It includes specifications on quality of materials, details on mixing and placing concrete, design assumptions for the analysis of continuous structures, and equations for proportioning members for design forces.All structures must be proportioned so they will not fail or deform excessively under any possible condition of service. Therefore it is important that an engineer use great care in anticipating all the probable loads to which a structure will be subjected during its lifetime.Although the design of most members is controlled typically by dead and live load acting simultaneously, consideration must also be given to the forces produced by wind, impact, shrinkage, temperature change, creep and support settlements, earthquake, and so forth.The load associated with the weight of the structure itself and its permanent components is called the dead load. The dead load of concrete members, which is substantial, should never be neglected in design computations. The exact magnitude of the dead load is not known accurately until members have been sized. Since some figure for the dead load must be used in computations to size the members, its magnitude must be estimated at first. After a structure has been analyzed, the members sized, and architectural details completed, the dead load can be computed more accurately. If the computed dead load is approximately equal to the initial estimate of its value ( or slightly less ), the design is complete, but if a significant difference exists between the computed and estimated values of dead weight, the computations should be revised using an improved value of dead load. An accurate estimate of dead load is particularly important when spans are long, say over 75 ft ( 22.9 m ),because dead load constitutes a major portion of the design load.Live loads associated with building use are specific items of equipment and occupants in a certain area of a building, building codes specify values of uniform live for which members are to be designed.After the structure has been sized for vertical load, it is checked for wind in combination with dead and live load as specified in the code. Wind loads do not usually control the size of members in building less than 16 to 18 stories, but for tall buildings wind loads become significant and cause large forces to develop in the structures. Under these conditions economy can be achieved only by selecting a structural system that is able to transfer horizontal loads into the ground efficiently.钢筋混凝土在每一个国家,混凝土及钢筋混凝土都被用来作为建筑材料。
(完整版)土木工程专业英语课文原文及对照翻译

Civil EngineeringCivil engineering, the oldest of the engineering specialties, is the planning, design, construction, and management of the built environment. This environment includes all structures built according to scientific principles, from irrigation and drainage systems to rocket-launching facilities.土木工程学作为最老的工程技术学科,是指规划,设计,施工及对建筑环境的管理。
此处的环境包括建筑符合科学规范的所有结构,从灌溉和排水系统到火箭发射设施。
Civil engineers build roads, bridges, tunnels, dams, harbors, power plants, water and sewage systems, hospitals, schools, mass transit, and other public facilities essential to modern society and large population concentrations. They also build privately owned facilities such as airports, railroads, pipelines, skyscrapers, and other large structures designed for industrial, commercial, or residential use. In addition, civil engineers plan, design, and build complete cities and towns, and more recently have been planning and designing space platforms to house self-contained communities.土木工程师建造道路,桥梁,管道,大坝,海港,发电厂,给排水系统,医院,学校,公共交通和其他现代社会和大量人口集中地区的基础公共设施。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
外文原文Response of a reinforced concrete infilled-frame structure to removal of twoadjacent columnsMehrdad Sasani_Northeastern University, 400 Snell Engineering Center, Boston, MA 02115, UnitedStatesReceived 27 June 2007; received in revised form 26 December 2007; accepted 24January 2008Available online 19 March 2008AbstractThe response of Hotel San Diego, a six-story reinforced concrete infilled-frame structure, is evaluated following the simultaneous removal of two adjacent exterior columns. Analytical models of the structure using the Finite Element Method as well as the Applied Element Method are used to calculate global and local deformations. The analytical results show good agreement with experimental data. The structure resisted progressive collapse with a measured maximum vertical displacement of only one quarter of an inch (6.4 mm). Deformation propagation over the height of the structure and the dynamic load redistribution following the column removal are experimentally and analytically evaluated and described. The difference between axial and flexural wave propagations is discussed. Three-dimensional Vierendeel (frame) action of the transverse and longitudinal frames with the participation of infill walls is identified as the major mechanism for redistribution of loads in the structure. The effects of two potential brittle modes of failure (fracture of beam sections without tensile reinforcement and reinforcing bar pull out) are described. The response of the structure due to additional gravity loads and in the absence of infill walls is analytically evaluated.c 2008 Elsevier Ltd. All rights reserved.Keywords: Progressive collapse; Load redistribution; Load resistance; Dynamic response; Nonlinear analysis; Brittle failure1.IntroductionThe principal scope of specifications is to provide general principles and computational methods in order to verify s afety of structures. The “safety factor ”, which according to modern trends is independent of the nature and combina tion of the materials used, can usually be defined as the ratio between the conditions. This ratio is also proportionalto the inverse of the probability ( risk ) of failure of the structure.Failure has to be considered not only as overall collapse of the structure but also as unserviceability or, according to a more precise. Common definition. As the reaching of a “limit state ”which causes the construction not to accomplish the task it was designed for. There are two ca tegories of limit state :(1)Ultimate limit sate, which corresponds to the highest value of the load-bearing capacity. Examples include local bu ckling or global instability of the structure; failure of so me sections and subsequent transformation of the structure in to a mechanism; failure by fatigue; elastic or plastic defor mation or creep that cause a substantial change of the geom etry of the structure; and sensitivity of the structure to alternating loads, to fire and to explosions.(2)Service limit states, which are functions of the use and durability of the structure. Examples include excessive d eformations and displacements without instability; early or ex cessive cracks; large vibrations; and corrosion.Computational methods used to verify structures with respe ct to the different safety conditions can be separated into:(1)Deterministic methods, in which the main parameters are considered as nonrandom parameters.(2)Probabilistic methods, in which the main parameters are considered as random parameters.Alternatively, with respect to the different use of facto rs of safety, computational methods can be separated into:(1)Allowable stress method, in which the stresses computed under maximum loads are compared with the strength of the material reduced by given safety factors.(2)Limit states method, in which the structure may be pr oportioned on the basis of its maximum strength. This streng th, as determined by rational analysis, shall not be less t han that required to support a factored load equal to the sum of the factored live load and dead load ( ultimate sta te ).The stresses corresponding to working ( service ) conditi ons with unfactored live and dead loads are compared with p rescribed values ( service limit state ) . From the four p ossible combinations of the first two and second two methods , we can obtain some useful computational methods. Generally, two combinations prevail:(1)deterministic methods, which make use of allowable stre sses. (2)Probabilistic methods, which make use of limit state s.The main advantage of probabilistic approaches is that, a t least in theory, it is possible to scientifically take in to account all random factors of safety, which are then com bined to define the safety factor. probabilistic approaches d epend upon :(1) Random distribution of strength of materials with res pect to the conditions of fabrication and erection ( scatter of the values of mechanical properties through out the str ucture ); (2) Uncertainty of the geometry of the cross-secti on sand of the structure ( faults and imperfections due to fabrication and erection of the structure );(3) Uncertainty of the predicted live loads and dead loa ds acting on the structure; (4)Uncertainty related to the ap proximation of the computational method used ( deviation of the actual stresses from computed stresses ). Furthermore, pr obabilistic theories mean that the allowable risk can be bas ed on several factors, such as :(1) Importance of the construction and gravity of the da mage by its failure; (2)Number of human lives which can be threatened by this failure; (3)Possibility and/or likelihood of repairing the structure; (4) Predicted life of the stru cture. All these factors are related to economic and social considerations such as:(1) Initial cost of the construction;(2) Amortization funds for the duration of the constructi on;(3) Cost of physical and material damage due to the fai lure of the construction;(4) Adverse impact on society;(5) Moral and psychological views.The definition of all these parameters, for a given safety factor, allows construction at the optimum cost. How ever, the difficulty of carrying out a complete probabilistic analysis has to be taken into account. For such an analys is the laws of the distribution of the live load and its induced stresses, of the scatter of mechanical properties of materials, and of the geometry of the cross-sections and t he structure have to be known. Furthermore, it is difficult to interpret the interaction between the law of distributio n of strength and that of stresses because both depend upon the nature of the material, on the cross-sections and upon the load acting on the structure. These practical difficult ies can be overcome in two ways. The first is to apply di fferent safety factors to the material and to the loads, without necessarily adopting the probabilistic criterion. The se cond is an approximate probabilistic method which introduces some simplifying assumptions ( semi-probabilistic methods ) . As part of mitigation programs to reduce the likelihood of mass casualties following local damage in structures, the General Services Administration [1] and the Department of Defense [2] developed regulations to evaluate progressive collapse resistance of structures. ASCE/SEI 7 [3] defines progressive collapse as the spread of an initial local failure from element to element eventually resulting in collapse of an entire structure or a disproportionately large part of it. Following the approaches proposed by Ellinwood and Leyendecker [4], ASCE/SEI 7 [3] defines two general methods for structural design of buildings to mitigate damage due to progressive collapse: indirect and direct design methods. General building codes and standards [3,5] use indirect design by increasing overall integrity of structures. Indirect design is also used in DOD [2]. Although the indirect design method can reduce the risk of progressive collapse [6,7] estimation of post-failure performance of structures designed based on such a method is not readily possible. One approach based on direct design methods to evaluate progressive collapse of structures is to study the effects of instantaneous removal of load-bearing elements, such as columns. GSA [1] and DOD [2] regulations require removal of one load bearing element. These regulations are meant to evaluate general integrity of structures and their capacity of redistributing the loads following severe damage to only one element. While such an approach provides insight as to the extent to which the structures are susceptible to progressive collapse, in reality, the initial damage can affect more than just one column. In this study, using analytical results that are verified against experimental data, the progressive collapse resistance of the Hotel San Diego is evaluated, following the simultaneous explosion (sudden removal) of two adjacent columns, one of which was a corner column. In order to explode the columns, explosives were inserted into predrilled holes in the columns. The columns were then well wrapped with a few layers of protective materials. Therefore, neither air blast nor flying fragments affected the structure.2. Building characteristicsHotel San Diego was constructed in 1914 with a south annex added in 1924. The annex included two separate buildings. Fig. 1 shows a south view of the hotel. Note that in the picture, the first and third stories of the hotel are covered with black fabric. The six story hotel had a non-ductile reinforced concrete (RC) frame structure with hollow clay tile exterior infill walls. The infills in the annex consisted of two withes (layers) of clay tiles with a total thickness of about 8 in (203 mm). The height of the first floor was about 190–800 (6.00 m). The height of other floors and that of the top floor were 100–600 (3.20 m) and 160–1000 (5.13 m), respectively. Fig. 2 shows the second floor of one of the annex buildings. Fig. 3 shows a typical plan of this building, whose response following the simultaneous removal (explosion) of columns A2 and A3 in the first (ground) floor is evaluated in this paper. The floor system consisted of one-way joists running in the longitudinal direction (North–South), as shown in Fig. 3. Based on compression tests of two concrete samples, the average concrete compressive strength was estimated at about 4500 psi (31 MPa) for a standard concrete cylinder. The modulus of elasticity of concrete was estimated at 3820 ksi (26 300 MPa) [5]. Also, based on tension tests of two steel samples having 1/2in (12.7 mm) square sections, the yield and ultimate tensile strengths were found to be 62 ksi (427 MPa) and 87 ksi (600 MPa), respectively. The steel ultimate tensile strain was measured at 0.17. The modulus of elasticity of steel was set equal to 29 000 ksi (200 000 MPa). The building was scheduled to be demolished by implosion. As part of the demolition process, the infill walls were removed from the first and third floors. There was no live load in the building. All nonstructural elements including partitions, plumbing, and furniture were removed prior to implosion. Only beams, columns, joist floor and infill walls on the peripheralbeams were present.3. SensorsConcrete and steel strain gages were used to measure changes in strains of beams and columns. Linear potentiometers were used to measure global and local deformations. The concrete strain gages were 3.5 in (90 mm) long having a maximum strain limit of ±0.02. The steel strain gages could measure up to a strain of ±0.20. The strain gages could operate up to a several hundred kHz sampling rate. The sampling rate used in the experiment was 1000 Hz. Potentiometers were used to capture rotation (integral of curvature over a length) of the beam end regions and global displacement in the building, as described later. The potentiometers had a resolution of about 0.0004 in (0.01 mm) and a maximum operational speed of about 40 in/s (1.0 m/s), while the maximum recorded speed in the experiment was about 14 in/s (0.35 m/s).4. Finite element modelUsing the finite element method (FEM), a model of the building was developed in the SAP2000 [8] computer program. The beams and columns are modeled with Bernoulli beam elements. Beams have T or L sections with effective flange width on each side of the web equal to four times the slab thickness [5]. Plastic hinges are assigned to all possible locations where steel bar yielding can occur, including the ends of elements as well as the reinforcing bar cut-off and bend locations. The characteristics of the plastic hinges are obtained using section analyses of the beams and columns and assuming a plastic hinge length equal to half of the section depth. The current version of SAP2000 [8] is not able to track formation of cracks in the elements. In order to find the proper flexural stiffness of sections, an iterative procedure is used as follows. First, the building is analyzed assuming all elements are uncracked. Then, moment demands in the elements are compared with theircracking bending moments, Mcr . The moment of inertia of beam and slab segments are reduced by a coefficient of 0.35 [5], where the demand exceeds the Mcr. The exterior beam cracking bending moments under negative and positive moments, are 516 k in (58.2 kN m) and 336 k in (37.9 kN m), respectively. Note that no cracks were formed in the columns. Then the building is reanalyzed and moment diagrams are re-evaluated. This procedure is repeated until all of the cracked regions are properly identified and modeled.The beams in the building did not have top reinforcing bars except at the end regions (see Fig. 4). For instance, no top reinforcement was provided beyond the bend in beam A1–A2, 12 inches away from the face of column A1 (see Figs. 4 and 5). To model the potential loss of flexural strength in those sections, localized crack hinges were assigned at the critical locations where no top rebar was present. Flexural strengths of the hinges were set equal to Mcr. Such sections were assumed to lose their flexural strength when the imposed bending moments reached Mcr.The floor system consisted of joists in the longitudinal direction (North–South). Fig. 6 shows the cross section of a typical floor. In order to account for potential nonlinear response of slabs and joists, floors are molded by beam elements. Joists are modeled with T-sections, having effective flange width on each side of the web equal to four times the slab thickness [5]. Given the large joist spacing between axes 2 and 3, two rectangular beam elements with 20-inch wide sections are used between the joist and the longitudinal beams of axes 2 and 3 to model the slab in the longitudinal direction. To model the behavior of theslab in the transverse direction, equally spaced parallel beams with 20-inch wide rectangular sections are used. There is a difference between the shear flow in the slab and that in the beam elements with rectangular sections modeling the slab. Because of this, the torsional stiffness is setequal to one-half of that of the gross sections [9].The building had infill walls on 2nd, 4th, 5th and 6th floors on the spandrel beams with some openings (i.e. windows and doors). As mentioned before and as part of the demolition procedure, the infill walls in the 1st and 3rd floors were removed before the test. The infill walls were made of hollow clay tiles, which were in good condition. The net area of the clay tiles was about 1/2 of the gross area. The in-plane action of the infill walls contributes to the building stiffness and strength and affects the building response. Ignoring the effects of the infill walls and excluding them in the model would result in underestimating the building stiffness and strength.Using the SAP2000 computer program [8], two types of modeling for the infills are considered in this study: one uses two dimensional shell elements (Model A) and the other uses compressive struts (Model B) as suggested in FEMA356 [10] guidelines.4.1. Model A (infills modeled by shell elements)Infill walls are modeled with shell elements. However, the current version of the SAP2000 computer program includes only linear shell elements and cannot account for cracking. The tensile strength of the infill walls is set equal to 26 psi, with a modulus of elasticity of 644 ksi [10]. Because the formation ofcracks has a significant effect on the stiffness of the infill walls, the following iterative procedure is used to account for crack formation:(1) Assuming the infill walls are linear and uncracked, a nonlinear time history analysis is run. Note that plastic hinges exist in the beam elements and the segments of the beam elements where moment demand exceeds the cracking moment have a reduced moment of inertia.(2) The cracking pattern in the infill wall is determined by comparing stresses in the shells developed during the analysis with the tensile strength of infills.(3) Nodes are separated at the locations where tensile stress exceeds tensile strength. These steps are continued until the crack regions are properly modeled.4.2. Model B (infills modeled by struts)Infill walls are replaced with compressive struts as described in FEMA 356 [10] guidelines. Orientations of the struts are determined from the deformed shape of the structure after column removal and the location of openings.4.3. Column removalRemoval of the columns is simulated with the following procedure.(1) The structure is analyzed under the permanent loads and the internal forces are determined at the ends of the columns, which will be removed.(2) The model is modified by removing columns A2 and A3 on the first floor. Again the structure is statically analyzed under permanent loads. In this case, the internal forces at the ends of removed columns found in the first step are applied externally to the structure along with permanent loads. Note that the results of this analysis are identical to those of step 1.(3) The equal and opposite column end forces that were applied in the second step are dynamically imposed on the ends of the removed column within one millisecond [11] to simulate the removal of the columns, and dynamic analysis is conducted.4.4. Comparison of analytical and experimental resultsThe maximum calculated vertical displacement of the building occurs at joint A3 in the second floor. Fig. 7 shows the experimental and analytical (Model A) vertical displacements of this joint (the AEM results will be discussed in the next section). Experimental data is obtained using the recordings of three potentiometers attached to joint A3 on one of their ends, and to the ground on the other ends. The peak displacements obtained experimentally and analytically (Model A) are 0.242 in (6.1 mm) and 0.252 in (6.4 mm), respectively, which differ only by about 4%. The experimental and analytical times corresponding to peak displacement are 0.069 s and 0.066 s, respectively. The analytical results show a permanent displacement of about 0.208 in (5.3 mm), which is about 14% smaller than the corresponding experimental value of 0.242 in (6.1 mm).Fig. 8 compares vertical displacement histories of joint A3 in the second floor estimated analytically based on Models A and B. As can be seen, modeling infills with struts (Model B) results in a maximum vertical displacement of joint A3 equal to about 0.45 in (11.4 mm), which is approximately 80% larger than the value obtained from Model A. Note that the results obtained from Model A are in close agreement with experimental results (see Fig. 7), while Model B significantly overestimates the deformation of the structure. If the maximum vertical displacement were larger, the infill walls were more severely cracked and the struts were more completely formed, the difference between the results of the two models (Models A and B) would be smaller.Fig. 9 compares the experimental and analytical (Model A) displacement of joint A2 in the second floor. Again, while the first peak vertical displacement obtained experimentally and analytically are in good agreement, the analytical permanent displacement under estimates the experimental value.Analytically estimated deformed shapes of the structure at the maximum vertical displacement based on Model A are shown in Fig. 10 with a magnification factor of 200. The experimentally measured deformed shape over the end regions of beams A1–A2 and A3–B3 in the second floorare represented in the figure by solid lines. A total of 14 potentiometers were located at the top and bottom of the end regions of the second floor beams A1–A2 and A3–B3, which were the most critical elements in load redistribution. The beam top and corresponding bottom potentiometer recordings were used to calculate rotation between the sections where the potentiometer ends were connected. This was done by first finding the difference between the recorded deformations at thetop and bottom of the beam, and then dividing the value by the distance (along the height of the beam section) between the two potentiometers. The expected deformed shapes between the measured end regions of the second floor beams are shown by dashed lines. As can be seen in the figures, analytically estimated deformed shapes of the beams are in good agreement with experimentally obtained deformed shapes.Analytical results of Model A show that only two plastic hinges are formed indicating rebar yielding. Also, four sections that did not have negative (top) reinforcement, reached cracking moment capacities and therefore cracked. Fig. 10 shows the locations of all the formed plastic hinges and cracks.。
