英文版罗斯公司理财习题答案Chap005

合集下载

英文版罗斯公司理财习题答案ChapWord版

CHAPTER 8MAKING CAPITAL INVESTMENT DECISIONSAnswers to Concepts Review and Critical Thinking Questions1.In this context, an opportunity cost refers to the value of an asset or other input that will be used in aproject. The relevant cost is what the asset or input is actually worth today, not, for example, what it cost to acquire.2. a.Yes, the reduction in the sales of the company’s other products, referred to as erosion, andshould be treated as an incremental cash flow. These lost sales are included because they are a cost (a revenue reduction) that the firm must bear if it chooses to produce the new product.b. Yes, expenditures on plant and equipment should be treated as incremental cash flows. Theseare costs of the new product line. However, if these expenditures have already occurred, they are sunk costs and are not included as incremental cash flows.c. No, the research and development costs should not be treated as incremental cash flows. Thecosts of research and development undertaken on the product during the past 3 years are sunk costs and should not be included in the evaluation of the project. Decisions made and costs incurred in the past cannot be changed. They should not affect the decision to accept or reject the project.d. Yes, the annual depreciation expense should be treated as an incremental cash flow.Depreciation expense must be taken into account when calculating the cash flows related to a given project. While depreciation is not a cash expense that directly affects cash flow, it decreases a firm’s net income and hence, lowers its tax bill for the year. Because of this depreciation tax shield, the firm has more cash on hand at the end of the year than it would have had without expensing depreciation.e.No, dividend payments should not be treated as incremental cash flows. A firm’s decision topay or not pay dividends is independent of the decision to accept or reject any given investment project. For this reason, it is not an incremental cash flow to a given project. Dividend policy is discussed in more detail in later chapters.f.Yes, the resale value of plant and equipment at the end of a project’s life should be treated as anincremental cash flow. The price at which the firm sells the equipment is a cash inflow, and any difference between the book value of the equipment and its sale price will create gains or lossesthat result in either a tax credit or liability.g.Yes, salary and medical costs for production employees hired for a project should be treated asincremental cash flows. The salaries of all personnel connected to the project must be included as costs of that project.3.Item I is a relevant cost because the opportunity to sell the land is lost if the new golf club is produced. Item II is also relevant because the firm must take into account the erosion of sales of existing products when a new product is introduced. If the firm produces the new club, the earnings from the existing clubs will decrease, effectively creating a cost that must be included in the decision.Item III is not relevant because the costs of Research and Development are sunk costs. Decisions made in the past cannot be changed. They are not relevant to the production of the new clubs.4.For tax purposes, a firm would choose MACRS because it provides for larger depreciationdeductions earlier. These larger deductions reduce taxes, but have no other cash consequences.Notice that the choice between MACRS and straight-line is purely a time value issue; the total depreciation is the same; only the timing differs.5.It’s probably only a mild over-simplification. Current liabilities will all be paid, presumably. Thecash portion of current assets will be retrieved. Some receivables won’t be collected, and some inventory will not be sold, of course. Counterbalancing these losses is the fact that inventory sold above cost (and not replaced at the end of the project’s life) acts to increase working capital. These effects tend to offset one another.6.Management’s discretion to set the firm’s capital structure is applicable at the firm level. Since anyone particular project could be financed entirely with equity, another project could be financed with debt, and the firm’s overall capital structure remains unchanged, financing cost s are not relevant in the analysis of a project’s incremental cash flows according to the stand-alone principle.7.The EAC approach is appropriate when comparing mutually exclusive projects with different livesthat will be replaced when they wear out. This type of analysis is necessary so that the projects havea common life span over which they can be compared; in effect, each project is assumed to existover an infinite horizon of N-year repeating projects. Assuming that this type of analysis is valid implies that the project cash flows remain the same forever, thus ignoring the possible effects of, among other things: (1) inflation, (2) changing economic conditions, (3) the increasing unreliability of cash flow estimates that occur far into the future, and (4) the possible effects of future technology improvement that could alter the project cash flows.8.Depreciation is a non-cash expense, but it is tax-deductible on the income statement. Thusdepreciation causes taxes paid, an actual cash outflow, to be reduced by an amount equal to the depreciation tax shield, t c D. A reduction in taxes that would otherwise be paid is the same thing as a cash inflow, so the effects of the depreciation tax shield must be added in to get the total incremental aftertax cash flows.9.There are two particularly important considerations. The first is erosion. Will the “essentialized”book simply displace copies of the existing book that would have otherwise been sold? This is of special concern given the lower price. The second consideration is competition. Will other publishers step in and produce such a product? If so, then any erosion is much less relevant. A particular concern to book publishers (and producers of a variety of other product types) is that the publisher only makes money from the sale of new books. Thus, it is important to examine whether the new book would displace sales of used books (good from the publisher’s perspective) or new books (not good). The concern arises any time there is an active market for used product.10.Definitely. The damage to Porsche’s reputation is definitely a factor the company needed to consider.If the reputation was damaged, the company would have lost sales of its existing car lines.11.One company may be able to produce at lower incremental cost or market better. Also, of course,one of the two may have made a mistake!12.Porsche would recognize that the outsized profits would dwindle as more products come to marketand competition becomes more intense.Solutions to Questions and ProblemsNOTE: All end-of-chapter problems were solved using a spreadsheet. Many problems require multiple steps. Due to space and readability constraints, when these intermediate steps are included in this solutions manual, rounding may appear to have occurred. However, the final answer for each problem is found without rounding during any step in the problem.Basicing the tax shield approach to calculating OCF, we get:OCF = (Sales – Costs)(1 – t C) + t C DepreciationOCF = [($5 × 2,000 – ($2 × 2,000)](1 – 0.35) + 0.35($10,000/5)OCF = $4,600So, the NPV of the project is:NPV = –$10,000 + $4,600(PVIFA17%,5)NPV = $4,7172.We will use the bottom-up approach to calculate the operating cash flow for each year. We also mustbe sure to include the net working capital cash flows each year. So, the total cash flow each year will be:Year 1 Year 2 Year 3 Year 4 Sales Rs.7,000 Rs.7,000 Rs.7,000 Rs.7,000Costs 2,000 2,000 2,000 2,000Depreciation 2,500 2,500 2,500 2,500EBT Rs.2,500 Rs.2,500 Rs.2,500 Rs.2,500Tax 850 850 850 850Net income Rs.1,650 Rs.1,650 Rs.1,650 Rs.1,650OCF 0 Rs.4,150 Rs.4,150 Rs.4,150 Rs.4,150Capital spending –Rs.10,000 0 0 0 0NWC –200 –250 –300 –200 950Incremental cashflow –Rs.10,200 Rs.3,900 Rs.3,850 Rs.3,950 Rs.5,100The NPV for the project is:NPV = –Rs.10,200 + Rs.3,900 / 1.10 + Rs.3,850 / 1.102 + Rs.3,950 / 1.103 + Rs.5,100 / 1.104NPV = Rs.2,978.333. Using the tax shield approach to calculating OCF, we get:OCF = (Sales – Costs)(1 – t C) + t C DepreciationOCF = (R2,400,000 – 960,000)(1 – 0.30) + 0.30(R2,700,000/3)OCF = R1,278,000So, the NPV of the project is:NPV = –R2,700,000 + R1,278,000(PVIFA15%,3)NPV = R217,961.704.The cash outflow at the beginning of the project will increase because of the spending on NWC. Atthe end of the project, the company will recover the NWC, so it will be a cash inflow. The sale of the equipment will result in a cash inflow, but we also must account for the taxes which will be paid on this sale. So, the cash flows for each year of the project will be:Year Cash Flow0 – R3,000,000 = –R2.7M – 300K1 1,278,0002 1,278,0003 1,725,000 = R1,278,000 + 300,000 + 210,000 + (0 – 210,000)(.30)And the NPV of the project is:NPV = –R3,000,000 + R1,278,000(PVIFA15%,2) + (R1,725,000 / 1.153)NPV = R211,871.465. First we will calculate the annual depreciation for the equipment necessary for the project. Thedepreciation amount each year will be:Year 1 depreciation = R2.7M(0.3330) = R899,100Year 2 depreciation = R2.7M(0.4440) = R1,198,800Year 3 depreciation = R2.7M(0.1480) = R399,600So, the book value of the equipment at the end of three years, which will be the initial investment minus the accumulated depreciation, is:Book value in 3 years = R2.7M – (R899,100 + 1,198,800 + 399,600)Book value in 3 years = R202,500The asset is sold at a gain to book value, so this gain is taxable.Aftertax salvage value = R202,500 + (R202,500 – 210,000)(0.30)Aftertax salvage value = R207,750To calculate the OCF, we will use the tax shield approach, so the cash flow each year is:OCF = (Sales – Costs)(1 – t C) + t C DepreciationYear Cash Flow0 – R3,000,000 = –R2.7M – 300K1 1,277,730.00 = (R1,440,000)(.70) + 0.30(R899,100)2 1,367,640.00 = (R1,440,000)(.70) + 0.30(R1,198,800)3 1,635,630.00 = (R1,440,000)(.70) + 0.30(R399,600) + R207,750 + 300,000Remember to include the NWC cost in Year 0, and the recovery of the NWC at the end of the project.The NPV of the project with these assumptions is:NPV = – R3.0M + (R1,277,730/1.15) + (R1,367,640/1.152) + (R1,635,630/1.153)NPV = R220,655.206. First, we will calculate the annual depreciation of the new equipment. It will be:Annual depreciation charge = €925,000/5Annual depreciation charge = €185,000The aftertax salvage value of the equipment is:Aftertax salvage value = €90,000(1 – 0.35)Aftertax salvage value = €58,500Using the tax shield approach, the OCF is:OCF = €360,000(1 – 0.35) + 0.35(€185,000)OCF = €298,750Now we can find the project IRR. There is an unusual feature that is a part of this project. Accepting this project means that we will reduce NWC. This reduction in NWC is a cash inflow at Year 0. This reduction in NWC implies that when the project ends, we will have to increase NWC. So, at the end of the project, we will have a cash outflow to restore the NWC to its level before the project. We also must include the aftertax salvage value at the end of the project. The IRR of the project is:NPV = 0 = –€925,000 + 125,000 + €298,750(PVIFA IRR%,5) + [(€58,500 – 125,000) / (1+IRR)5]IRR = 23.85%7.First, we will calculate the annual depreciation of the new equipment. It will be:Annual depreciation = £390,000/5Annual depreciation = £78,000Now, we calculate the aftertax salvage value. The aftertax salvage value is the market price minus (or plus) the taxes on the sale of the equipment, so:Aftertax salvage value = MV + (BV – MV)t cVery often, the book value of the equipment is zero as it is in this case. If the book value is zero, the equation for the aftertax salvage value becomes:Aftertax salvage value = MV + (0 – MV)t cAftertax salvage value = MV(1 – t c)We will use this equation to find the aftertax salvage value since we know the book value is zero. So, the aftertax salvage value is:Aftertax salvage value = £60,000(1 – 0.34)Aftertax salvage value = £39,600Using the tax shield approach, we find the OCF for the project is:OCF = £120,000(1 – 0.34) + 0.34(£78,000)OCF = £105,720Now we can find the project NPV. Notice that we include the NWC in the initial cash outlay. The recovery of the NWC occurs in Year 5, along with the aftertax salvage value.NPV = –£390,000 – 28,000 + £105,720(PVIFA10%,5) + [(£39,600 + 28,000) / 1.15]NPV = £24,736.268.To find the BV at the end of four years, we need to find the accumulated depreciation for the firstfour years. We could calculate a table with the depreciation each year, but an easier way is to add the MACRS depreciation amounts for each of the first four years and multiply this percentage times the cost of the asset. We can then subtract this from the asset cost. Doing so, we get:BV4 = $9,300,000 – 9,300,000(0.2000 + 0.3200 + 0.1920 + 0.1150)BV4 = $1,608,900The asset is sold at a gain to book value, so this gain is taxable.Aftertax salvage value = $2,100,000 + ($1,608,900 – 2,100,000)(.40)Aftertax salvage value = $1,903,5609. We will begin by calculating the initial cash outlay, that is, the cash flow at Time 0. To undertake theproject, we will have to purchase the equipment and increase net working capital. So, the cash outlay today for the project will be:Equipment –€2,000,000NWC –100,000Total –€2,100,000Using the bottom-up approach to calculating the operating cash flow, we find the operating cash flow each year will be:Sales €1,200,000Costs 300,000Depreciation 500,000EBT €400,000Tax 140,000Net income €260,000The operating cash flow is:OCF = Net income + DepreciationOCF = €260,000 + 500,000OCF = €760,000To find the NPV of the project, we add the present value of the project cash flows. We must be sure to add back the net working capital at the end of the project life, since we are assuming the net working capital will be recovered. So, the project NPV is:NPV = –€2,100,000 + €760,000(PVIFA14%,4) + €100,000 / 1.144NPV = €173,629.3810.We will need the aftertax salvage value of the equipment to compute the EAC. Even though theequipment for each product has a different initial cost, both have the same salvage value. The aftertax salvage value for both is:Both cases: aftertax salvage value = $20,000(1 – 0.35) = $13,000To calculate the EAC, we first need the OCF and NPV of each option. The OCF and NPV for Techron I is:OCF = – $34,000(1 – 0.35) + 0.35($210,000/3) = $2,400NPV = –$210,000 + $2,400(PVIFA14%,3) + ($13,000/1.143) = –$195,653.45EAC = –$195,653.45 / (PVIFA14%,3) = –$84,274.10And the OCF and NPV for Techron II is:OCF = – $23,000(1 – 0.35) + 0.35($320,000/5) = $7,450NPV = –$320,000 + $7,450(PVIFA14%,5) + ($13,000/1.145) = –$287,671.75EAC = –$287,671.75 / (PVIFA14%,5) = –$83,794.05The two milling machines have unequal lives, so they can only be compared by expressing both on an equivalent annual basis, which is what the EAC method does. Thus, you prefer the Techron II because it has the lower (less negative) annual cost.。

《公司理财》课后答案(英文版,第六版).doc

Chapter 2: Accounting Statements and Cash Flow2.10AssetsCurrent assetsCash $ 4,000Accounts receivable 8,000Total current assets $ 12,000Fixed assetsMachinery $ 34,000Patents 82,000Total fixed assets $116,000Total assets $128,000Liabilities and equityCurrent liabilitiesAccounts payable $ 6,000Taxes payable 2,000Total current liabilities $ 8,000Long-term liabilitiesBonds payable $7,000Stockholders equityCommon stock ($100 par) $ 88,000Capital surplus 19,000Retained earnings 6,000Total stockholders equity $113,000Total liabilities and equity $128,0002.11One year ago TodayLong-term debt $50,000,000 $50,000,000Preferred stock 30,000,000 30,000,000Common stock 100,000,000 110,000,000Retained earnings 20,000,000 22,000,000Total $200,000,000 $212,000,0002.12Total Cash Flow ofthe Stancil CompanyCash flows from the firmCapital spending $(1,000)Additions to working capital (4,000)Total $(5,000)Cash flows to investors of the firmShort-term debt $(6,000)Long-term debt (20,000)Equity (Dividend - Financing) 21,000Total $(5,000)[Note: This table isn’t the Statement of Cash Flows, which is only covered in Appendix 2B, since the latter has th e change in cash (on the balance sheet) as a final entry.]2.13 a. The changes in net working capital can be computed from:Sources of net working capitalNet income $100Depreciation 50Increases in long-term debt 75Total sources $225Uses of net working capitalDividends $50Increases in fixed assets* 150Total uses $200Additions to net working capital $25*Includes $50 of depreciation.b.Cash flow from the firmOperating cash flow $150Capital spending (150)Additions to net working capital (25)Total $(25)Cash flow to the investorsDebt $(75)Equity 50Total $(25)Chapter 3: Financial Markets and Net Present Value: First Principles of Finance (Advanced)3.14 $120,000 - ($150,000 - $100,000) (1.1) = $65,0003.15 $40,000 + ($50,000 - $20,000) (1.12) = $73,6003.16 a. ($7 million + $3 million) (1.10) = $11.0 millionb.i. They could spend $10 million by borrowing $5 million today.ii. They will have to spend $5.5 million [= $11 million - ($5 million x 1.1)] at t=1.Chapter 4: Net Present Valuea. $1,000 ⨯ 1.0510 = $1,628.89b. $1,000 ⨯ 1.0710 = $1,967.15c. $1,000 ⨯ 1.0520 = $2,653.30d. Interest compounds on the interest already earned. Therefore, the interest earned inSince this bond has no interim coupon payments, its present value is simply the present value of the $1,000 that will be received in 25 years. Note: As will be discussed in the next chapter, the present value of the payments associated with a bond is the price of that bond.PV = $1,000 /1.125 = $92.30PV = $1,500,000 / 1.0827 = $187,780.23a. At a discount rate of zero, the future value and present value are always the same. Remember, FV =PV (1 + r) t. If r = 0, then the formula reduces to FV = PV. Therefore, the values of the options are $10,000 and $20,000, respectively. You should choose the second option.b. Option one: $10,000 / 1.1 = $9,090.91Option two: $20,000 / 1.15 = $12,418.43Choose the second option.c. Option one: $10,000 / 1.2 = $8,333.33Option two: $20,000 / 1.25 = $8,037.55Choose the first option.d. You are indifferent at the rate that equates the PVs of the two alternatives. You know that rate mustfall between 10% and 20% because the option you would choose differs at these rates. Let r be thediscount rate that makes you indifferent between the options.$10,000 / (1 + r) = $20,000 / (1 + r)5(1 + r)4 = $20,000 / $10,000 = 21 + r = 1.18921r = 0.18921 = 18.921%The $1,000 that you place in the account at the end of the first year will earn interest for six years. The $1,000 that you place in the account at the end of the second year will earn interest for five years, etc. Thus, the account will have a balance of$1,000 (1.12)6 + $1,000 (1.12)5 + $1,000 (1.12)4 + $1,000 (1.12)3= $6,714.61PV = $5,000,000 / 1.1210 = $1,609,866.18a. $1.000 (1.08)3 = $1,259.71b. $1,000 [1 + (0.08 / 2)]2 ⨯ 3 = $1,000 (1.04)6 = $1,265.32c. $1,000 [1 + (0.08 / 12)]12 ⨯ 3 = $1,000 (1.00667)36 = $1,270.24d. $1,000 e0.08 ⨯ 3 = $1,271.25e. The future value increases because of the compounding. The account is earning interest on interest. Essentially, the interest is added to the account balance at the e nd of every compounding period. During the next period, the account earns interest on the new balance. When the compounding period shortens, the balance that earns interest is rising faster.The price of the consol bond is the present value of the coupon payments. Apply the perpetuity formula to find the present value. PV = $120 / 0.15 = $800a. $1,000 / 0.1 = $10,000b. $500 / 0.1 = $5,000 is the value one year from now of the perpetual stream. Thus, the value of theperpetuity is $5,000 / 1.1 = $4,545.45.c. $2,420 / 0.1 = $24,200 is the value two years from now of the perpetual stream. Thus, the value of the perpetuity is $24,200 / 1.12 = $20,000.pply the NPV technique. Since the inflows are an annuity you can use the present value of an annuity factor.ANPV = -$6,200 + $1,200 81.0= -$6,200 + $1,200 (5.3349)= $201.88Yes, you should buy the asset.Use an annuity factor to compute the value two years from today of the twenty payments. Remember, the annuity formula gives you the value of the stream one year before the first payment. Hence, the annuity factor will give you the value at the end of year two of the stream of payments.A= $2,000 (9.8181)Value at the end of year two = $2,000 20.008= $19,636.20The present value is simply that amount discounted back two years.PV = $19,636.20 / 1.082 = $16,834.88The easiest way to do this problem is to use the annuity factor. The annuity factor must be equal to $12,800 / $2,000 = 6.4; remember PV =C A T r. The annuity factors are in the appendix to the text. To use the factor table to solve this problem, scan across the row labeled 10 years until you find 6.4. It is close to the factor for 9%, 6.4177. Thus, the rate you will receive on this note is slightly more than 9%.You can find a more precise answer by interpolating between nine and ten percent.[ 10% ⎤[6.1446 ⎤a ⎡r ⎥bc ⎡6.4 ⎪ d⎣9%⎦⎣6.4177 ⎦By interpolating, you are presuming that the ratio of a to b is equal to the ratio of c to d.(9 - r ) / (9 - 10) = (6.4177 - 6.4 ) / (6.4177 - 6.1446)r = 9.0648%The exact value could be obtained by solving the annuity formula for the interest rate. Sophisticated calculators can compute the rate directly as 9.0626%.[Note: A standard financial calculator’s TVM keys can solve for this rate. With annuity flows, the IRR key on “advanced” financial c alculators is unnecessary.]a. The annuity amount can be computed by first calculating the PV of the $25,000 which youThat amount is $17,824.65 [= $25,000 / 1.075]. Next compute the annuity which has the same present value.A$17,824.65 = C 507.0$17,824.65 = C (4.1002)C = $4,347.26Thus, putting $4,347.26 into the 7% account each year will provide $25,000 five years from today.b. The lump sum payment must be the present value of the $25,000, i.e., $25,000 / 1.075 =$17,824.65The formula for future value of any annuity can be used to solve the problem (see footnote 11 of the text).Option one: This cash flow is an annuity due. To value it, you must use the after-tax amounts. Theafter-tax payment is $160,000 (1 - 0.28) = $115,200. Value all except the first payment using the standard annuity formula, then add back the first payment of $115,200 to obtain the value of this option.AValue = $115,200 + $115,200 30.010= $115,200 + $115,200 (9.4269)= $1,201,178.88Option two: This option is valued similarly. You are able to have $446,000 now; this is already on an after-tax basis. You will receive an annuity of $101,055 for each of the next thirty years. Those payments are taxable when you receive them, so your after-tax payment is $72,759.60 [= $101,055 (1 - 0.28)].AValue = $446,000 + $72,759.60 30.010= $446,000 + $72,759.60 (9.4269)= $1,131,897.47Since option one has a higher PV, you should choose it.et r be the rate of interest you must earn.$10,000(1 + r)12 = $80,000(1 + r)12= 8r = 0.18921 = 18.921%First compute the present value of all the payments you must make for your children’s educati on. The value as of one year before matriculation of one child’s education isA= $21,000 (2.8550) = $59,955.$21,000 415.0This is the value of the elder child’s education fourteen years from now. It is the value of the younger child’s education sixteen years from today. The present value of these isPV = $59,955 / 1.1514 + $59,955 / 1.1516= $14,880.44You want to make fifteen equal payments into an account that yields 15% so that the present value of the equal payments is $14,880.44.A= $14,880.44 / 5.8474 = $2,544.80Payment = $14,880.44 / 15.015This problem applies the growing annuity formula. The first payment is$50,000(1.04)2(0.02) = $1,081.60.PV = $1,081.60 [1 / (0.08 - 0.04) - {1 / (0.08 - 0.04)}{1.04 / 1.08}40]= $21,064.28This is the present value of the payments, so the value forty years from today is$21,064.28 (1.0840) = $457,611.46se the discount factors to discount the individual cash flows. Then compute the NPV of the project. NoticeYou can still use the factor tables to compute their PV. Essentially, they form cash flows that are a six year annuity less a two year annuity. Thus, the appropriate annuity factor to use with them is 2.6198 (= 4.3553 - 1.7355).Year Cash Flow Factor PV0.9091 $636.371$70020.8264 743.769003 1,000 ⎤4 1,000 ⎥ 2.6198 2,619.805 1,000 ⎥6 1,000 ⎦7 1,250 0.5132 641.508 1,375 0.4665 641.44Total $5,282.87NPV = -$5,000 + $5,282.87= $282.87Purchase the machine.Chapter 5: How to Value Bonds and StocksThe amount of the semi-annual interest payment is $40 (=$1,000 ⨯ 0.08 / 2). There are a total of 40 periods;i.e., two half years in each of the twenty years in the term to maturity. The annuity factor tables can be usedto price these bonds. The appropriate discount rate to use is the semi-annual rate. That rate is simply the annual rate divided by two. Thus, for part b the rate to be used is 5% and for part c is it 3%.A+F/(1+r)40PV=C Tra. $40 (19.7928) + $1,000 / 1.0440 = $1,000Notice that whenever the coupon rate and the market rate are the same, the bond is priced at par.b. $40 (17.1591) + $1,000 / 1.0540 = $828.41Notice that whenever the coupon rate is below the market rate, the bond is priced below par.c. $40 (23.1148) + $1,000 / 1.0340 = $1,231.15Notice that whenever the coupon rate is above the market rate, the bond is priced above par.a. The semi-annual interest rate is $60 / $1,000 = 0.06. Thus, the effective annual rate is 1.062 - 1 =0.1236 = 12.36%.A+ $1,000 / 1.0612b. Price = $30 12.006= $748.48A+ $1,000 / 1.0412c. Price = $30 1204.0= $906.15Note: In parts b and c we are implicitly assuming that the yield curve is flat. That is, the yield in year 5applies for year 6 as well.rice = $2 (0.72) / 1.15 + $4 (0.72) / 1.152 + $50 / 1.153= $36.31The number of shares you own = $100,000 / $36.31 = 2,754 sharesPrice = $1.15 (1.18) / 1.12 + $1.15 (1.182) / 1.122 + $1.152 (1.182) / 1.123+ {$1.152 (1.182)(1.06) / (0.12 - 0.06)} / 1.123= $26.95[Insert before last sentence of question: Assume that dividends are a fixed proportion of earnings.] Dividend one year from now = $5 (1 - 0.10) = $4.50Price = $5 + $4.50 / {0.14 - (-0.10)}= $23.75Since the current $5 dividend has not yet been paid, it is still included in the stock price.Chapter 6: Some Alternative Investment Rulesa. Payback period of Project A = 1 + ($7,500 - $4,000) / $3,500 = 2 yearsPayback period of Project B = 2 + ($5,000 - $2,500 -$1,200) / $3,000 = 2.43 yearsProject A should be chosen.b. NPV A = -$7,500 + $4,000 / 1.15 + $3,500 / 1.152 + $1,500 / 1.153 = -$388.96NPV B = -$5,000 + $2,500 / 1.15 + $1,200 / 1.152 + $3,000 / 1.153 = $53.83Project B should be chosen.a. Average Investment:($16,000 + $12,000 + $8,000 + $4,000 + 0) / 5 = $8,000Average accounting return:$4,500 / $8,000 = 0.5625 = 56.25%b. 1. AAR does not consider the timing of the cash flows, hence it does not consider the timevalue of money.2. AAR uses an arbitrary firm standard as the decision rule.3. AAR uses accounting data rather than net cash flows.aAverage Investment = (8000 + 4000 + 1500 + 0)/4 = 3375.00Average Net Income = 2000(1-0.75) = 1500=> AAR = 1500/3375=44.44%a. Solve x by trial and error:-$8,000 + $4,000 / (1 + x) + $3000 / (1 + x)2 + $2,000 / (1 + x)3 = 0x = 6.93%b. No, since the IRR (6.93%) is less than the discount rate of 8%.Alternatively, the NPV @ a discount rate of 0.08 = -$136.62.a. Solve r in the equation:$5,000 - $2,500 / (1 + r) - $2,000 / (1 + r)2 - $1,000 / (1 + r)3- $1,000 / (1 + r)4 = 0By trial and error,IRR = r = 13.99%b. Since this problem is the case of financing, accept the project if the IRR is less than the required rate of return.IRR = 13.99% > 10%Reject the offer.c. IRR = 13.99% < 20%Accept the offer.d. When r = 10%:NPV = $5,000 - $2,500 / 1.1 - $2,000 / 1.12 - $1,000 / 1.13 - $1,000 / 1.14When r = 20%:NPV = $5,000 - $2,500 / 1.2 - $2,000 / 1.22 - $1,000 / 1.23 - $1,000 / 1.24= $466.82Yes, they are consistent with the choices of the IRR rule since the signs of the cash flows change only once.A/ $160,000 = 1.04PI = $40,000 715.0Since the PI exceeds one accept the project.Chapter 7: Net Present Value and Capital BudgetingSince there is uncertainty surrounding the bonus payments, which McRae might receive, you must use the expected value of McRae’s bonuses in the computation of the PV of his contract. McRae’s salary plus the expected value of his bonuses in years one through three is$250,000 + 0.6 ⨯ $75,000 + 0.4 ⨯ $0 = $295,000.Thus the total PV of his three-year contract isPV = $400,000 + $295,000 [(1 - 1 / 1.12363) / 0.1236]+ {$125,000 / 1.12363} [(1 - 1 / 1.123610 / 0.1236]= $1,594,825.68EPS = $800,000 / 200,000 = $4NPVGO = (-$400,000 + $1,000,000) / 200,000 = $3Price = EPS / r + NPVGO= $4 / 0.12 + $3=$36.33Year 0 Year 1 Year 2 Year 3 Year 4 Year 51. Annual Salary$120,000 $120,000 $120,000 $120,000 $120,000 Savings2. Depreciation 100,000 160,000 96,000 57,600 57,6003. Taxable Income 20,000 -40,000 24,000 62,400 62,4004. Taxes 6,800 -13,600 8,160 21,216 21,2165. Operating Cash Flow113,200 133,600 111,840 98,784 98,784 (line 1-4)$100,000 -100,0006. ∆ Net workingcapital7. Investment $500,000 75,792*8. Total Cash Flow -$400,000 $113,200 $133,600 $111,840 $98,784 $74,576*75,792 = $100,000 - 0.34 ($100,000 - $28,800)NPV = -$400,000+ $113,200 / 1.12 + $133,600 / 1.122 + $111,840 / 1.123+ $98,784 / 1.124 + $74,576 / 1.125= -$7,722.52Real interest rate = (1.15 / 1.04) - 1 = 10.58%NPV A = -$40,000+ $20,000 / 1.1058 + $15,000 / 1.10582 + $15,000 / 1.10583= $1,446.76NPV B = -$50,000+ $10,000 / 1.15 + $20,000 / 1.152 + $40,000 / 1.153= $119.17Choose project A.PV = $120,000 / {0.11 - (-0.06)}t = 0 t = 1 t = 2 t = 3 t = 4 t = 5 t = 6 ...$12,000 $6,000 $6,000 $6,000$4,000$12,000 $6,000 $6,000 ...The present value of one cycle is:A+ $4,000 / 1.064PV = $12,000 + $6,000 306.0= $12,000 + $6,000 (2.6730) + $4,000 / 1.064= $31,206.37The cycle is four years long, so use a four year annuity factor to compute the equivalent annual cost (EAC).AEAC = $31,206.37 / 406.0= $31,206.37 / 3.4651= $9,006The present value of such a stream in perpetuity is$9,006 / 0.06 = $150,100o evaluate the word processors, compute their equivalent annual costs (EAC).BangAPV(costs) = (10 ⨯ $8,000) + (10 ⨯ $2,000) 414.0= $80,000 + $20,000 (2.9137)= $138,274EAC = $138,274 / 2.9137= $47,456IOUAPV(costs) = (11 ⨯ $5,000) + (11 ⨯ $2,500) 3.014- (11 ⨯ $500) / 1.143= $55,000 + $27,500 (2.3216) - $5,500 / 1.143= $115,132EAC = $115,132 / 2.3216= $49,592BYO should purchase the Bang word processors.Chapter 8: Strategy and Analysis in Using Net Present ValueThe accounting break-even= (120,000 + 20,000) / (1,500 - 1,100)= 350 units. The accounting break-even= 340,000 / (2.00 - 0.72)= 265,625 abalonesb. [($2.00 ⨯ 300,000) - (340,000 + 0.72 ⨯ 300,000)] (0.65)= $28,600This is the after tax profit.Chapter 9: Capital Market Theory: An Overviewa. Capital gains = $38 - $37 = $1 per shareb. Total dollar returns = Dividends + Capital Gains = $1,000 + ($1*500) = $1,500 On a per share basis, this calculation is $2 + $1 = $3 per sharec. On a per share basis, $3/$37 = 0.0811 = 8.11% On a total dollar basis, $1,500/(500*$37) = 0.0811 = 8.11%d. No, you do not need to sell the shares to include the capital gains in the computation of the returns. The capital gain is included whether or not you realize the gain. Since you could realize the gain if you choose, you should include it.The expected holding period return is:()[]%865.1515865.052$/52$75.54$50.5$==-+There appears to be a lack of clarity about the meaning of holding period returns. The method used in the answer to this question is the one used in Section 9.1. However, the correspondence is not exact, because in this question, unlike Section 9.1, there are cash flows within the holding period. The answer above ignores the dividend paid in the first year. Although the answer above technically conforms to the eqn at the bottom of Fig. 9.2, the presence of intermediate cash flows that aren’t accounted for renders th is measure questionable, at best. There is no similar example in the body of the text, and I have never seen holding period returns calculated in this way before.Although not discussed in this book, there are two generally accepted methods of computing holding period returns in the presence of intermediate cash flows. First, the time weighted return calculates averages (geometric or arithmetic) of returns between cash flows. Unfortunately, that method can’t be used here, because we are not given the va lue of the stock at the end of year one. Second, the dollar weighted measure calculates the internal rate of return over the entire holding period. Theoretically, that method can be applied here, as follows: 0 = -52 + 5.50/(1+r) + 60.25/(1+r)2 => r = 0.1306.This produces a two year holding period return of (1.1306)2 – 1 = 0.2782. Unfortunately, this book does not teach the dollar weighted method.In order to salvage this question in a financially meaningful way, you would need the value of the stock at the end of one year. Then an illustration of the correct use of the time-weighted return would be appropriate. A complicating factor is that, while Section 9.2 illustrates the holding period return using the geometric return for historical data, the arithmetic return is more appropriate for expected future returns.E(R) = T-Bill rate + Average Excess Return = 6.2% + (13.0% -3.8%) = 15.4%. Common Treasury Realized Stocks Bills Risk Premium -7 32.4% 11.2% 21.2%-6 -4.9 14.7 -19.6-5 21.4 10.5 10.9 -4 22.5 8.8 13.7 -3 6.3 9.9 -3.6 -2 32.2 7.7 24.5 Last 18.5 6.2 12.3 b. The average risk premium is 8.49%.49.873.125.246.37.139.106.192.21=++-++- c. Yes, it is possible for the observed risk premium to be negative. This can happen in any single year. The.b.Standard deviation = 03311.0001096.0=.b.Standard deviation = = 0.03137 = 3.137%.b.Chapter 10: Return and Risk: The Capital-Asset-Pricing Model (CAPM)a. = 0.1 (– 4.5%) + 0.2 (4.4%) + 0.5 (12.0%) + 0.2 (20.7%) = 10.57%b.σ2 = 0.1 (–0.045 – 0.1057)2 + 0.2 (0.044 – 0.1057)2 + 0.5 (0.12 – 0.1057)2+ 0.2 (0.207 – 0.1057)2 = 0.0052σ = (0.0052)1/2 = 0.072 = 7.20%Holdings of Atlas stock = 120 ⨯ $50 = $6,000 ⨯ $20 = $3,000Weight of Atlas stock = $6,000 / $9,000 = 2 / 3Weight of Babcock stock = $3,000 / $9,000 = 1 / 3a. = 0.3 (0.12) + 0.7 (0.18) = 0.162 = 16.2%σP 2= 0.32 (0.09)2 + 0.72 (0.25)2 + 2 (0.3) (0.7) (0.09) (0.25) (0.2)= 0.033244σP= (0.033244)1/2 = 0.1823 = 18.23%a.State Return on A Return on B Probability1 15% 35% 0.4 ⨯ 0.5 = 0.22 15% -5% 0.4 ⨯ 0.5 = 0.23 10% 35% 0.6 ⨯ 0.5 = 0.34 10% -5% 0.6 ⨯ 0.5 = 0.3b. = 0.2 [0.5 (0.15) + 0.5 (0.35)] + 0.2[0.5 (0.15) + 0.5 (-0.05)]+ 0.3 [0.5 (0.10) + 0.5 (0.35)] + 0.3 [0.5 (0.10) + 0.5 (-0.05)]= 0.135= 13.5%Note: The solution to this problem requires calculus.Specifically, the solution is found by minimizing a function subject to a constraint. Calculus ability is not necessary to understand the principles behind a minimum variance portfolio.Min { X A2 σA2 + X B2σB2+ 2 X A X B Cov(R A , R B)}subject to X A + X B = 1Let X A = 1 - X B. Then,Min {(1 - X B)2σA2 + X B2σB2+ 2(1 - X B) X B Cov (R A, R B)}Take a derivative with respect to X B.d{∙} / dX B = (2 X B - 2) σA2+ 2 X B σB2 + 2 Cov(R A, R B) - 4 X B Cov(R A, R B)Set the derivative equal to zero, cancel the common 2 and solve for X B.X BσA2- σA2+ X B σB2 + Cov(R A, R B) - 2 X B Cov(R A, R B) = 0X B = {σA2 - Cov(R A, R B)} / {σA2+ σB2 - 2 Cov(R A, R B)}andX A = {σB2 - Cov(R A, R B)} / {σA2+ σB2 - 2 Cov(R A, R B)}Using the data from the problem yields,X A = 0.8125 andX B = 0.1875.a. Using the weights calculated above, the expected return on the minimum variance portfolio isE(R P) = 0.8125 E(R A) + 0.1875 E(R B)= 0.8125 (5%) + 0.1875 (10%)= 5.9375%b. Using the formula derived above, the weights areX A = 2 / 3 andX B = 1 / 3c. The variance of this portfolio is zero.σP 2= X A2 σA2 + X B2σB2+ 2 X A X B Cov(R A , R B)= (4 / 9) (0.01) + (1 / 9) (0.04) + 2 (2 / 3) (1 / 3) (-0.02)= 0This demonstrates that assets can be combined to form a risk-free portfolio.14.2%= 3.7%+β(7.5%) ⇒β = 1.40.25 = R f + 1.4 [R M– R f] (I)0.14 = R f + 0.7 [R M– R f] (II)(I) – (II)=0.11 = 0.7 [R M– R f] (III)[R M– R f ]= 0.1571Put (III) into (I) 0.25 = R f + 1.4[0.1571]R f = 3%[R M– R f ]= 0.1571R M = 0.1571 + 0.03= 18.71%a. = 4.9% + βi (9.4%)βD= Cov(R D, R M) / σM 2 = 0.0635 / 0.04326 = 1.468= 4.9 + 1.468 (9.4) = 18.70%Weights:X A = 5 / 30 = 0.1667X B = 10 / 30 = 0.3333X C = 8 / 30 = 0.2667X D = 1 - X A - X B - X C = 0.2333Beta of portfolio= 0.1667 (0.75) + 0.3333 (1.10) + 0.2667 (1.36) + 0.2333 (1.88)= 1.293= 4 + 1.293 (15 - 4) = 18.22%a. (i) βA= ρA,MσA / σMρA,M= βA σM / σA= (0.9) (0.10) / 0.12= 0.75(ii) σB= βB σM / ρB,M= (1.10) (0.10) / 0.40= 0.275(iii) βC= ρC,MσC / σM= (0.75) (0.24) / 0.10= 1.80(iv) ρM,M= 1(v) βM= 1(vi) σf= 0(vii) ρf,M= 0(viii) βf= 0b. SML:E(R i) = R f + βi {E(R M) - R f}= 0.05 + (0.10) βiSecurity βi E(R i)A 0.13 0.90 0.14B 0.16 1.10 0.16C 0.25 1.80 0.23Security A performed worse than the market, while security C performed better than the market.Security B is fairly priced.c. According to the SML, security A is overpriced while security C is under-priced. Thus, you could invest in security C while sell security A (if you currently hold it).a. The typical risk-averse investor seeks high returns and low risks. To assess thetwo stocks, find theReturns:State of economy ProbabilityReturn on A*Recession 0.1 -0.20 Normal 0.8 0.10 Expansion0.10.20* Since security A pays no dividend, the return on A is simply (P 1 / P 0) - 1. = 0.1 (-0.20) + 0.8 (0.10) + 0.1 (0.20) = 0.08 = 0.09 This was given in the problem.Risk:R A - (R A -)2 P ⨯ (R A -)2 -0.28 0.0784 0.00784 0.02 0.0004 0.00032 0.12 0.0144 0.00144 Variance 0.00960Standard deviation (R A ) = 0.0980βA = {Corr(R A , R M ) σ(R A )} / σ(R M ) = 0.8 (0.0980) / 0.10= 0.784βB = {Corr(R B , R M ) σ(R B )} / σ(R M ) = 0.2 (0.12) / 0.10= 0.24The return on stock B is higher than the return on stock A. The risk of stock B, as measured by itsbeta, is lower than the risk of A. Thus, a typical risk-averse investor will prefer stock B.b. = (0.7) + (0.3) = (0.7) (0.8) + (0.3) (0.09) = 0.083σP 2= 0.72 σA 2 + 0.32 σB 2 + 2 (0.7) (0.3) Corr (R A , R B ) σA σB = (0.49) (0.0096) + (0.09) (0.0144) + (0.42) (0.6) (0.0980) (0.12) = 0.0089635 σP = = 0.0947 c. The beta of a portfolio is the weighted average of the betas of the components of the portfolio. βP = (0.7) βA + (0.3) βB = (0.7) (0.784) + (0.3) (0.240) = 0.621Chapter 11:An Alternative View of Risk and Return: The Arbitrage Pricing Theorya. Stock A:()()R R R R R A A A m m Am A=+-+=+-+βεε105%12142%...Stock B:()()R R R R R B B m m Bm B=+-+=+-+βεε130%098142%...Stock C:()R R R R R C C C m m Cm C=+-+=+-+βεε157%137142%)..(.b.()[]()[]()[]()()()()()()[]()()CB A m cB A m c m B m A m CB A P 25.045.030.0%2.14R 1435.1%925.1225.045.030.0%2.14R 37.125.098.045.02.130.0%7.1525.0%1345.0%5.1030.0%2.14R 37.1%7.1525.0%2.14R 98.0%0.1345.0%2.14R 2.1%5.1030.0R 25.0R 45.0R 30.0R ε+ε+ε+-+=ε+ε+ε+-+++++=ε+-++ε+-++ε+-+=++= c.i.()R R R A B C =+-==+-==+-=105%1215%142%)1113%09815%142%)137%157%13715%142%168%..(..46%.(......ii.R P =+-=12925%1143515%142%)138398%..(..To determine which investment investor would prefer, you must compute the variance of portfolios created bymany stocks from either market. Note, because you know that diversification is good, it is reasonable to assume that once an investor chose the market in which he or she will invest, he or she will buy many stocks in that market.Known:E EF ====001002 and and for all i.i σσεε..Assume: The weight of each stock is 1/N; that is, X N i =1/for all i.If a portfolio is composed of N stocks each forming 1/N proportion of the portfolio, the return on the portfolio is 1/N times the sum of the returns on the N stocks. Recall that the return on each stock is 0.1+βF+ε.()()()()()()[]()()()()()()()[]()[]()[]()()[]()()()()()j i 2j i 22j i i 2222222222P P P P iP ,0.04Corr 0.01,Cov s =isvariance the ,N as limit In the ,Cov 1/N 1s 1/N s )(1/N 1/N F 2F E 1/N F E 0.10.1/N F 0.1E R E R E R Var 0.101/N 00.1E 1/N F E 0.11/N F 0.1E R E 1/N F 0.1F 0.1(1/N)R 1/N R εε+β=εε+β∞⇒εε-+ε+β=ε∑+εβ+β=ε+β=-ε+β+=-==+β+=ε+β+=ε∑+β+=ε+β+=ε+β+==∑∑∑∑∑∑∑∑()()()()()()Thus,F R f E R E R Var R Corr Var R Corr ii ip P p i j PijR 1i =++=++===+=+010*********002250040002500412212111222.........,,εεεεεεa.()()()()Corr Corr Var R Var R i j i j p pεεεε112212000225000225,,..====Since Var ()()R p 1 Var R 2p 〉, a risk averse investor will prefer to invest in the second market.b. Corr ()()εεεε112090i j j ,.,== and Corr 2i()()Var R Var R pp120058500025==..。

(公司理财)英文版罗斯公司理财习题答案C

CHAPTER 20INTERNATIONAL CORPORATE FINANCEAnswers to Concepts Review and Critical Thinking Questions1. a.The dollar is selling at a premium because it is more expensive in the forward market than inthe spot market (SFr 1.53 versus SFr 1.50).b.The franc is expected to depreciate relative to the dollar because it will take more francs to buyone dollar in the future than it does today.c.Inflation in Switzerland is higher than in the United States, as are nominal interest rates.2.The exchange rate will increase, as it will take progressively more pesos to purchase a dollar. This isthe relative PPP relationship.3. a.The Australian dollar is expected to weaken relative to the dollar, because it will take moreA$ in the future to buy one dollar than it does today.b.The inflation rate in Australia is higher.c.Nominal interest rates in Australia are higher; relative real rates in the two countries are thesame.4. A Yankee bond is most accurately described by d.5. No. For example, if a country’s currency strengthens, imports become cheaper (good), but its exportsbecome more expensive for others to buy (bad). The reverse is true for currency depreciation.6.Additional advantages include being closer to the final consumer and, thereby, saving ontransportation, significantly lower wages, and less exposure to exchange rate risk. Disadvantages include political risk and costs of supervising distant operations.7.One key thing to remember is that dividend payments are made in the home currency. Moregenerally, it may be that the owners of the multinational are primarily domestic and are ultimately concerned about their wealth denominated in their home currency because, unlike a multinational, they are not internationally diversified.8. a.False. If prices are rising faster in Great Britain, it will take more pounds to buy the sameamount of goods that one dollar can buy; the pound will depreciate relative to the dollar.b.False. The forward market would already reflect the projected deterioration of the euro relativeto the dollar. Only if you feel that there might be additional, unanticipated weakening of the euro that isn’t reflected in forward rates today, will the forward hedge protect you against additional declines.c.True. The market would only be correct on average, while you would be correct all the time.9. a.American exporters: their situation in general improves because a sale of the exported goods fora fixed number of euros will be worth more dollars.American importers: their situation in general worsens because the purchase of the imported goods for a fixed number of euros will cost more in dollars.b.American exporters: they would generally be better off if the British government’s intentionsresult in a strengthened pound.American importers: they would generally be worse off if the pound strengthens.c.American exporters: they would generally be much worse off, because an extreme case of fiscalexpansion like this one will make American goods prohibitively expensive to buy, or else Brazilian sales, if fixed in cruzeiros, would become worth an unacceptably low number of dollars.American importers: they would generally be much better off, because Brazilian goods will become much cheaper to purchase in dollars.10.IRP is the most likely to hold because it presents the easiest and least costly means to exploit anyarbitrage opportunities. Relative PPP is least likely to hold since it depends on the absence of market imperfections and frictions in order to hold strictly.11.It all depends on whether the forward market expects the same appreciation over the period andwhether the expectation is accurate. Assuming that the expectation is correct and that other traders do not have the same information, there will be value to hedging the currency exposure.12.One possible reason investment in the foreign subsidiary might be preferred is if this investmentprovides direct diversification that shareholders could not attain by investing on their own. Another reason could be if the political climate in the foreign country was more stable than in the home country. Increased political risk can also be a reason you might prefer the home subsidiary investment. Indonesia can serve as a great example of political risk. If it cannot be diversified away, investing in this type of foreign country will increase the systematic risk. As a result, it will raise the cost of the capital, and could actually decrease the NPV of the investment.13.Yes, the firm should undertake the foreign investment. If, after taking into consideration all risks, aproject in a foreign country has a positive NPV, the firm should undertake it. Note that in practice, the stated assumption (that the adjustment to the discount rate has taken into consideration all political and diversification issues) is a huge task. But once that has been addressed, the net present value principle holds for foreign operations, just as for domestic.14.If the foreign currency depreciates, the U.S. parent will experience an exchange rate loss when theforeign cash flow is remitted to the U.S. This problem could be overcome by selling forward contracts. Another way of overcoming this problem would be to borrow in the country where the project is located.15.False. If the financial markets are perfectly competitive, the difference between the Eurodollar rateand the U.S. rate will be due to differences in risk and government regulation. Therefore, speculating in those markets will not be beneficial.16.The difference between a Eurobond and a foreign bond is that the foreign bond is denominated in thecurrency of the country of origin of the issuing company. Eurobonds are more popular than foreign bonds because of registration differences. Eurobonds are unregistered securities.Solutions to Questions and ProblemsNOTE: All end-of-chapter problems were solved using a spreadsheet. Many problems require multiple steps. Due to space and readability constraints, when these intermediate steps are included in this solutions manual, rounding may appear to have occurred. However, the final answer for each problem is found without rounding during any step in the problem.Basicing the quotes from the table, we get:a.$50(€0.7870/$1) = €39.35b.$1.2706c.€5M($1.2706/€) = $6,353,240d.New Zealand dollare.Mexican pesof.(P11.0023/$1)($1.2186/€1) = P13.9801/€This is a cross rate.g.The most valuable is the Kuwait dinar. The least valuable is the Indonesian rupiah.2. a.You would prefer £100, since:(£100)($.5359/£1) = $53.59b.You would still prefer £100. Using the $/£ exchange rate and the SF/£ exchange rate to find theamount of Swiss francs £100 will buy, we get:(£100)($1.8660/£1)(SF .8233) = SF 226.6489ing the quotes in the book to find the SF/£ cross rate, we find:(SF 1.2146/$1)($0.5359/£1) = SF 2.2665/£1The £/SF exchange rate is the inverse of the SF/£ exchange rate, so:£1/SF .4412 = £0.4412/SF 13. a.F180= ¥104.93 (per $). The yen is selling at a premium because it is more expensive in theforward market than in the spot market ($0.0093659 versus $0.009530).b.F90 = $1.8587/£. The pound is selling at a discount because it is less expensive in the forwardmarket than in the spot market ($0.5380 versus $0.5359).c.The value of the dollar will fall relative to the yen, since it takes more dollars to buy one yen inthe future than it does today. The value of the dollar will rise relative to the pound, because it will take fewer dollars to buy one pound in the future than it does today.4. a.The U.S. dollar, since one Canadian dollar will buy:(Can$1)/(Can$1.26/$1) = $0.7937b.The cost in U.S. dollars is:(Can$2.19)/(Can$1.26/$1) = $1.74Among the reasons that absolute PPP doe sn’t hold are tariffs and other barriers to trade, transactions costs, taxes, and different tastes.c.The U.S. dollar is selling at a discount, because it is less expensive in the forward market thanin the spot market (Can$1.22 versus Can$1.26).d.The Canadian dollar is expected to appreciate in value relative to the dollar, because it takesfewer Canadian dollars to buy one U.S. dollar in the future than it does today.e.Interest rates in the United States are probably higher than they are in Canada.5. a.The cross rate in ¥/£ terms is:(¥115/$1)($1.70/£1) = ¥195.5/£1b.The yen is quoted too low relative to the pound. Take out a loan for $1 and buy ¥115. Use the¥115 to purchase pounds at the cross-rate, which will give you:¥115(£1/¥185) = £0.6216Use the pounds to buy back dollars and repay the loan. The cost to repay the loan will be:£0.6216($1.70/£1) = $1.0568You arbitrage profit is $0.0568 per dollar used.6.We can rearrange the interest rate parity condition to answer this question. The equation we will useis:R FC = (F T– S0)/S0 + R USUsing this relationship, we find:Great Britain: R FC = (£0.5394 – £0.5359)/£0.5359 + .038 = 4.45%Japan: R FC = (¥104.93 – ¥106.77)/¥106.77 + .038 = 2.08%Switzerland: R FC = (SFr 1.1980 – SFr 1.2146)/SFr 1.2146 + .038 = 2.43%7.If we invest in the U.S. for the next three months, we will have:$30M(1.0045)3 = $30,406,825.23If we invest in Great Britain, we must exchange the dollars today for pounds, and exchange the pounds for dollars in three months. After making these transactions, the dollar amount we would have in three months would be:($30M)(£0.56/$1)(1.0060)3/(£0.59/$1) = $28,990,200.05We should invest in U.S.ing the relative purchasing power parity equation:F t = S0 × [1 + (h FC– h US)]tWe find:Z3.92 = Z3.84[1 + (h FC– h US)]3h FC– h US = (Z3.92/Z3.84)1/3– 1h FC– h US = .0069Inflation in Poland is expected to exceed that in the U.S. by 0.69% over this period.9.The profit will be the quantity sold, times the sales price minus the cost of production. Theproduction cost is in Singapore dollars, so we must convert this to U.S. dollars. Doing so, we find that if the exchange rates stay the same, the profit will be:Profit = 30,000[$145 – {(S$168.50)/(S$1.6548/$1)}]Profit = $1,295,250.18If the exchange rate rises, we must adjust the cost by the increased exchange rate, so:Profit = 30,000[$145 – {(S$168.50)/1.1(S$1.6548/$1)}]Profit = $1,572,954.71If the exchange rate falls, we must adjust the cost by the decreased exchange rate, so:Profit = 30,000[$145 – {(S$168.50)/0.9(S$1.6548/$1)}]Profit = $955,833.53To calculate the breakeven change in the exchange rate, we need to find the exchange rate that make the cost in Singapore dollars equal to the selling price in U.S. dollars, so:$145 = S$168.50/S TS T = S$1.1621/$1S T = –.2978 or –29.78% decline10. a.If IRP holds, then:F180 = (Kr 6.43)[1 + (.08 – .05)]1/2F180 = Kr 6.5257Since given F180 is Kr6.56, an arbitrage opportunity exists; the forward premium is too high.Borrow Kr1 today at 8% interest. Agree to a 180-day forward contract at Kr 6.56. Convert the loan proceeds into dollars:Kr 1 ($1/Kr 6.43) = $0.15552Invest these dollars at 5%, ending up with $0.15931. Convert the dollars back into krone as$0.15931(Kr 6.56/$1) = Kr 1.04506Repay the Kr 1 loan, ending with a profit of:Kr1.04506 – Kr1.03868 = Kr 0.00638b.To find the forward rate that eliminates arbitrage, we use the interest rate parity condition, so:F180 = (Kr 6.43)[1 + (.08 – .05)]1/2F180 = Kr 6.525711.The international Fisher effect states that the real interest rate across countries is equal. We canrearrange the international Fisher effect as follows to answer this question:R US– h US = R FC– h FCh FC = R FC + h US– R USa.h AUS = .05 + .035 – .039h AUS = .046 or 4.6%b.h CAN = .07 + .035 – .039h CAN = .066 or 6.6%c.h TAI = .10 + .035 – .039h TAI = .096 or 9.6%12. a.The yen is expected to get stronger, since it will take fewer yen to buy one dollar in the futurethan it does today.b.h US– h JAP (¥129.76 – ¥131.30)/¥131.30h US– h JAP = – .0117 or –1.17%(1 – .0117)4– 1 = –.0461 or –4.61%The approximate inflation differential between the U.S. and Japan is – 4.61% annually.13. We need to find the change in the exchange rate over time, so we need to use the relative purchasingpower parity relationship:F t = S0 × [1 + (h FC– h US)]TUsing this relationship, we find the exchange rate in one year should be:F1 = 215[1 + (.086 – .035)]1F1 = HUF 225.97The exchange rate in two years should be:F2 = 215[1 + (.086 – .035)]2F2 = HUF 237.49And the exchange rate in five years should be:F5 = 215[1 + (.086 – .035)]5F5 = HUF 275.71ing the interest-rate parity theorem:(1 + R US) / (1 + R FC) = F(0,1) / S0We can find the forward rate as:F(0,1) = [(1 + R US) / (1 + R FC)] S0F(0,1) = (1.13 / 1.08)$1.50/£F(0,1) = $1.57/£Intermediate15.First, we need to forecast the future spot rate for each of the next three years. From interest rate andpurchasing power parity, the expected exchange rate is:E(S T) = [(1 + R US) / (1 + R FC)]T S0So:E(S1) = (1.0480 / 1.0410)1 $1.22/€ = $1.2282/€E(S2) = (1.0480 / 1.0410)2 $1.22/€ = $1.2365/€E(S3) = (1.0480 / 1.0410)3 $1.22/€ = $1.2448/€Now we can use these future spot rates to find the dollar cash flows. The dollar cash flow each year will be:Year 0 cash flow = –€$12,000,000($1.22/€) = –$14,640,000.00Year 1 cash flow = €$2,700,000($1.2282/€) = $3,316,149.86Year 2 cash flow = €$3,500,000($1.2365/€) = $4,327,618.63Year 3 cash flow = (€3,300,000 + 7,400,000)($1.2448/€) = $13,319,111.90And the NPV of the project will be:NPV = –$14,640,000 + $3,316,149.86/1.13 + $4,4327,618.63/1.132 + $13,319,111.90/1.133NPV = $914,618.7316. a.Implicitly, it is assumed that interest rates won’t change over the life of the project, but theexchange rate is projected to decline because the Euroswiss rate is lower than the Eurodollar rate.b.We can use relative purchasing power parity to calculate the dollar cash flows at each time. Theequation is:E[S T] = (SFr 1.72)[1 + (.07 – .08)]TE[S T] = 1.72(.99)TSo, the cash flows each year in U.S. dollar terms will be:t SFr E[S T] US$0 –27.0M –$15,697,674.421 +7.5M 1.7028 $4,404,510.222 +7.5M 1.6858 $4,449,000.223 +7.5M 1.6689 $4,493,939.624 +7.5M 1.6522 $4,539,332.955 +7.5M 1.6357 $4,585,184.79And the NPV is:NPV = –$15,697,674.42 + $4,404,510.22/1.13 + $4,449,000.22/1.132 + $4,493,939.62/1.133 + $4,539,332.95/1.134 + $4,585,184.79/1.135NPV = $71,580.10c.Rearranging the relative purchasing power parity equation to find the required return in Swissfrancs, we get:R SFr = 1.13[1 + (.07 – .08)] – 1R SFr = 11.87%So, the NPV in Swiss francs is:NPV = –SFr 27.0M + SFr 7.5M(PVIFA11.87%,5)NPV = SFr 123,117.76Converting the NPV to dollars at the spot rate, we get the NPV in U.S. dollars as:NPV = (SFr 123,117.76)($1/SFr 1.72)NPV = $71,580.10Challenge17. a.The domestic Fisher effect is:1 + R US = (1 + r US)(1 + h US)1 + r US = (1 + R US)/(1 + h US)This relationship must hold for any country, that is:1 + r FC = (1 + R FC)/(1 + h FC)The international Fisher effect states that real rates are equal across countries, so:1 + r US = (1 + R US)/(1 + h US) = (1 + R FC)/(1 + h FC) = 1 + r FCb.The exact form of unbiased interest rate parity is:E[S t] = F t = S0 [(1 + R FC)/(1 + R US)]tc.The exact form for relative PPP is:E[S t] = S0 [(1 + h FC)/(1 + h US)]td.For the home currency approach, we calculate the expected currency spot rate at time t as:E[S t] = (€0.5)[1.07/1.05]t= (€0.5)(1.019)tWe then convert the euro cash flows using this equation at every time, and find the present value. Doing so, we find:NPV = –[€2M/(€0.5)] + {€0.9M/[1.019(€0.5)]}/1.1 + {€0.9M/[1.0192(€0.5)]}/1.12 + {€0.9M/[1.0193(€0.5/$1)]}/1.13NPV = $316,230.72For the foreign currency approach, we first find the return in the euros as:R FC = 1.10(1.07/1.05) – 1 = 0.121Next, we find the NPV in euros as:NPV = –€2M + (€0.9M)/1.121 + (€0.9M)/1.1212+ (€0.9M)/1.1213= €158,115.36And finally, we convert the euros to dollars at the current exchange rate, which is:NPV ($) = €158,115.36 /(€0.5/$1) = $316,230.72。

罗斯-公司理财-英文练习题-附带答案-第九章

罗斯-公司理财-英文练习题-附带答案-第九章CHAPTER 9Risk Analysis, Real Options, and Capital Budgeting Multiple Choice Questions:I. DEFINITIONSSCENARIO ANALYSISb 1. An analysis of what happens to the estimate of the net present value when you examinea number of different likely situations is called _____ analysis.a. forecastingb. scenarioc. sensitivityd. simulatione. break-evenDifficulty level: EasySENSITIVITY ANALYSISc 2. An analysis of what happens to the estimate of net present value when only onevariable is changed is called _____ analysis.a. forecastingb. scenarioc. sensitivityd. simulatione. break-evenDifficulty level: EasySIMULATION ANALYSISd 3. An analysis which combines scenario analysis with sensitivity analysis is called _____analysis.a. forecastingb. scenarioc. sensitivityd. simulatione. break-evenDifficulty level: EasyBREAK-EVEN ANALYSISe 4. An analysis of the relationship between the sales volume and various measures ofprofitability is called _____ analysis.a. forecastingb. scenarioc. sensitivityd. simulatione. break-evenDifficulty level: EasyVARIABLE COSTSa 5. Variable costs:a. change in direct relationship to the quantity of output produced.b. are constant in the short-run regardless of the quantity of output produced.c. reflect the change in a variable when one more unit of output is produced.d. are subtracted from fixed costs to compute the contribution margin.e. form the basis that is used to determine the degree of operating leverage employed by afirm.Difficulty level: EasyFIXED COSTSb 6. Fixed costs:a. change as the quantity of output produced changes.b. are constant over the short-run regardless of the quantity of output produced.c. reflect the change in a variable when one more unit of output is produced.d. are subtracted from sales to compute the contribution margin.e. can be ignored in scenario analysis since they are constant over the life of a project.Difficulty level: EasyACCOUNTING BREAK-EVENc 7. The sales level that results in a project’s net income exactly equaling zero is called the_____ break-even.a. operationalb. leveragedc. accountingd. cashe. present valueDifficulty level: EasyPRESENT VALUE BREAK-EVENe 8. The sales level that results in a project’s net present value exactly equaling zero iscalled the _____ break-even.a. operationalb. leveragedc. accountingd. cashe. present valueDifficulty level: EasyII. CONCEPTSSCENARIO ANALYSISb 9. Conducting scenario analysis helps managers see the:a. impact of an individual variable on the outcome of a project.b. potential range of outcomes from a proposed project.c. changes in long-term debt over the course of a proposed project.d. possible range of market prices for their stock over the life of a project.e. allocation distribution of funds for capital projects under conditions of hard rationing.Difficulty level: EasySENSITIVITY ANALYSISb 10. Sensitivity analysis helps you determine the:a. range of possible outcomes given possible ranges for every variable.b. degree to which the net present value reacts to changes in a single variable.c. net present value given the best and the worst possible situations.d. degree to which a project is reliant upon the fixed costs.e. level of variable costs in relation to the fixed costs of a project.Difficulty level: EasySENSITIVITY ANALYSISc 11. As the degree of sensitivity of a project to a single variable rises, the:a. lower the forecasting risk of the project.b. smaller the range of possible outcomes given a pre-defined range of values for theinput.c. more attention management should place on accurately forecasting the future value ofthat variable.d. lower the maximum potential value of the project.e. lower the maximum potential loss of the project.Difficulty level: MediumSENSITIVITY ANALYSISc 12. Sensitivity analysis is conducted by:a. holding all variables at their base level and changing the required rate of returnassigned to a project.b. changing the value of two variables to determine their interdependency.c. changing the value of a single variable and computing the resulting change in thecurrent value of a project.d. assigning either the best or the worst possible value to each variable and comparing theresults to those achieved by the base case.e. managers after a project has been implemented to determine how each variable relatesto the level of output realized.Difficulty level: MediumSENSITIVITY ANALYSISd 13. To ascertain whether the accuracy of the variable cost estimate for a project will havemuch effect on the final outcome of the project, you should probably conduct _____analysis.a. leverageb. scenarioc. break-evend. sensitivitye. cash flowDifficulty level: EasySIMULATIONd 14. Simulation analysis is based on assigning a _____ and analyzing the results.a. narrow range of values to a single variableb. narrow range of values to multiple variables simultaneouslyc. wide range of values to a single variabled. wide range of values to multiple variables simultaneouslye. single value to each of the variablesDifficulty level: MediumSIMULATIONe 15. The type of analysis that is most dependent upon the use of a computer is _____analysis.a. scenariob. break-evenc. sensitivityd. degree of operating leveragee. simulationDifficulty level: EasyVARIABLE COSTSd 16. Which one of the following is most likely a variable cost?a. office rentb. property taxesc. property insuranced. direct labor costse. management salariesDifficulty level: EasyVARIABLE COSTSa 17. Which of the following statements concerning variable costs is (are) correct?I. Variable costs minus fixed costs equal marginal costs.II. Variable costs are equal to zero when production is equal to zero.III. An increase in variable costs increases the operating cash flow.a. II onlyb. III onlyc. I and III onlyd. II and III onlye. I and II onlyDifficulty level: MediumVARIABLE COSTSa 18. All else constant, as the variable cost per unit increases, the:a. contribution margin decreases.b. sensitivity to fixed costs decreases.c. degree of operating leverage decreases.d. operating cash flow increases.e. net profit increases.Difficulty level: MediumFIXED COSTSc 19. Fixed costs:I. are variable over long periods of time.II. must be paid even if production is halted.III. are generally affected by the amount of fixed assets owned by a firm.IV. per unit remain constant over a given range of production output.a. I and III onlyb. II and IV onlyc. I, II, and III onlyd. I, II, and IV onlye. I, II, III, and IVDifficulty level: MediumCONTRIBUTION MARGINc 20. The contribution margin must increase as:a. both the sales price and variable cost per unit increase.b. the fixed cost per unit declines.c. the gap between the sales price and the variable cost per unit widens.d. sales price per unit declines.e. the sales price minus the fixed cost per unit increases.Difficulty level: MediumACCOUNTING BREAK-EVENa 21. Which of the following statements are correct concerning the accounting break-evenpoint?I. The net income is equal to zero at the accounting break-even point.II. The net present value is equal to zero at the accounting break-even point.III. The quantity sold at the accounting break-even point is equal to the total fixed costs plus depreciation divided by the contribution margin.IV. The quantity sold at the accounting break-even point is equal to the total fixed costs divided by the contribution margin.a. I and III onlyb. I and IV onlyc. II and III onlyd. II and IV onlye. I, II, and IV onlyDifficulty level: MediumACCOUNTING BREAK-EVENb 22. All else constant, the accounting break-even level of sales will decrease when the:a. fixed costs increase.b. depreciation expense decreases.c. contribution margin decreases.d. variable costs per unit increase.e. selling price per unit decreases.Difficulty level: MediumPRESENT VALUE BREAK-EVENd 23. The point where a project produces a rate of return equal to the required return isknown as the:a. point of zero operating leverage.b. internal break-even point.c. accounting break-even point.d. present value break-even point.e. internal break-even point.Difficulty level: EasyPRESENT VALUE BREAK-EVENb 24. Which of the following statements are correct concerning the present value break-evenpoint of a project?I. The present value of the cash inflows equals the amount of the initial investment.II. The payback period of the project is equal to the life of the project.III. The operating cash flow is at a level that produces a net present value of zero.IV. The project never pays back on a discounted basis.a. I and II onlyb. I and III onlyc. II and IV onlyd. III and IV onlye. I, III, and IV onlyDifficulty level: MediumINVESTMENT TIMING DECISIONb 25. The investment timing decision relates to:a. how long the cash flows last once a project is implemented.b. the decision as to when a project should be started.c. how frequently the cash flows of a project occur.d. how frequently the interest on the debt incurred to finance a project is compounded.e. the decision to either finance a project over time or pay out the initial cost in cash.Difficulty level: MediumOPTION TO WAITe 26. The timing option that gives the option to wait:I. may be of minimal value if the project relates to a rapidly changing technology.II. is partially dependent upon the discount rate applied to the project being evaluated.III. is defined as the situation where operations are shut down for a period of time.IV. has a value equal to the net present value of the project if it is started today versus the net present value if it is started at some later date.a. I and III onlyb. II and IV onlyc. I and II onlyd. II, III, and IV onlye. I, II, and IV onlyDifficulty level: ChallengeOPTION TO EXPANDb 27. Last month you introduced a new product to the market. Consumer demand has beenoverwhelming and appears that strong demand will exist over the long-term. Given thissituation, management should consider the option to:a. suspend.b. expand.c. abandon.d. contract.e. withdraw.Difficulty level: EasyOPTION TO EXPANDc 28. Including the option to expand in your project analysis will tend to:a. extend the duration of a project but not affect the project’s net present value.b. incre ase the cash flows of a project but decrease the project’s net present value.c. increase the net present value of a project.d. decrease the net present value of a project.e. have no effect on either a project’s c ash flows or its net present value.Difficulty level: MediumSENSITIVITY AND SENARIO ANALYSISd 29. Theoretically, the NPV is the most appropriate method to determine the acceptabilityof a project. A false sense of security can be overwhelm the decision-maker when theprocedure is applied properly and the positive NPV resultsare accepted blindly.Sensitivity and scenario analysis aid in the process bya. changing the underlying assumptions on which the decision is based.b. highlights the areas where more and better data are needed.c. providing a picture of how an event can affect the calculations.d. All of the above.e. None of the above.Difficulty level: MediumDECSION TREEa 30. In order to make a decision with a decision treea. one starts farthest out in time to make the first decision.b. one must begin at time 0.c. any path can be taken to get to the end.d. any path can be taken to get back to the beginning.e. None of the above.Difficulty level: MediumDECISION TREEc 31. In a decision tree, the NPV to make the yes/no decision is dependent ona. only the cash flows from successful path.b. on the path where the probabilities add up to one.c. all cash flows and probabilities.d. only the cash flows and probabilities of the successful path.e. None of the above.Difficulty level: MediumDECISION TREEe 32. In a decision tree, caution should be used in analysis becausea. early stage decisions are probably riskier and should not likely use the same discountrate.b. if a negative NPV is actually occurring, management should opt out of the project andminimize their loss.c. decision trees are only used for planning, not actually daily management.d. Both A and C.e. Both A and B.Difficulty level: MediumSENSITIVITY ANALYSISd 33. Sensitivity analysis evaluates the NPV with respect toa. changes in the underlying assumptions.b. one variable changing while holding the others constant.c. different economic conditions.d. All of the above.e. None of the above.Difficulty level: MediumSENSITIVITY ANALYSISd 34. Sensitivity analysis provides information ona. whether the NPV should be trusted, it may provide a false sense of security if allNPVs are positive.b. the need for additional information as it tests each variablein isolation.c. the degree of difficulty in changing multiple variables together.d. Both A and B.e. Both A and C.Difficulty level: MediumFIXED COSTSb 35. Fixed production costs area. directly related to labor costs.b. measured as cost per unit of time.c. measured as cost per unit of output.d. dependent on the amount of goods or services produced.e. None of the above.Difficulty level: MediumVARIABLE COSTSd 36. Variable costsa. change as the quantity of output changes.b. are zero when production is zero.c. are exemplified by direct labor and raw materials.d. All of the above.e. None of the above.Difficulty level: EasySENSITIVITY ANALYSISb 37. An investigation of the degree to which NPV depends on assumptions made about anysingular critical variable is called a(n)a. operating analysis.b. sensitivity analysis.c. marginal benefit analysis.d. decision tree analysis.e. None of the above.Difficulty level: EasySENSITIVITY AND SCENARIOS ANALYSISb 38. Scenario analysis is different than sensitivity analysisa. as no economic forecasts are changed.b. as several variables are changed together.c. because scenario analysis deals with actual data versus sensitivity analysis which dealswith a forecast.d. because it is short and simple.e. because it is 'by the seat of the pants' technique.Difficulty level: MediumEQUIVALENT ANNUAL COSTc 39. In the present-value break-even the EAC is used toa. determine the opportunity cost of investment.b. allocate depreciation over the life of the project.c. allocate the initial investment at its opportunity cost over the life of the project.d. determine the contribution margin to fixed costs.e. None of the above.Difficulty level: MediumBREAK-EVENb 40. The present value break-even point is superior to the accounting break-even pointbecausea. present value break-even is more complicated to calculate.b. present value break-even covers the economic opportunity costs of the investment.c. present value break-even is the same as sensitivity analysis.d. present value break-even covers the fixed costs of production, which the accountingbreak-even does not.e. present value break-even covers the variable costs of production, which the accountingbreak-even does not.Difficulty level: EasyABANDONMENTd 41. The potential decision to abandon a project has option value becausea. abandonment can occur at any future point in time.b. a project may be worth more dead than alive.c. management is not locked into a negative outcome.d. All of the above.e. None of the above.Difficulty level: EasyTYPES OF BREAK-EVEN ANALYSISd 42. Which of the following are types of break-even analysis?a. present value break-evenb. accounting profit break-evenc. market value break-evend. Both A and B.e. Both A and C.Difficulty level: EasyMONTE CARLO SIMULATIONc 43. The approach that further attempts to model real word uncertainty by analyzingprojects the way one might analyze gambling strategies is calleda. gamblers approach.b. blackjack approach.c. Monte Carlo simulation.d. scenario analysis.e. sensitivity analysis.Difficulty level: MediumMONTE CARLO SIMULATIONc 44. Monte Carlo simulation isa. the most widely used by executives.b. a very simple formula.c. provides a more complete analysis that sensitivity or scenario.d. the oldest capital budgeting technique.e. None of the above.Difficulty level: EasyOPTIONS IN CAPITAL BUDGETINGd 45. Which of the following are hidden options in capital budgeting?a. option to expand.b. timing option.c. option to abandon.d. All of the above.e. None of the above.Difficulty level: EasyIII. PROBLEMSUse this information to answer questions 46 through 50.The Adept Co. is analyzing a proposed project. The company expects to sell 2,500units, give or take 10 percent. The expected variable cost per unit is $8 and the expected fixed costs are $12,500. Cost estimates are considered accurate within a plus or minus 5 percent range. The depreciation expense is $4,000. The sale price is estimated at $16 aunit, give or take 2 percent. The company bases their sensitivity analysis on the expected case scenario.SCENARIO ANALYSISd 46. What is the sales revenue under the optimistic case scenario?a. $40,000b. $43,120c. $44,000d. $44,880e. $48,400Difficulty level: MediumSCENARIO ANALYSISd 47. What is the contribution margin under the expected case scenario?a. $2.67b. $3.00c. $7.92d. $8.00e. $8.72Difficulty level: MediumSCENARIO ANALYSISc 48. What is the amount of the fixed cost per unit under the pessimistic case scenario?a. $4.55b. $5.00c. $5.83d. $6.02e. $6.55Difficulty level: MediumSENSITIVITY ANALYSISb 49. The company is conducting a sensitivity analysis on the sales price using a salesprice estimate of $17. Using this value, the earnings before interest and taxes will be:a. $4,000b. $6,000c. $8,500d. $10,000e. $18,500Difficulty level: MediumSENSITIVITY ANALYSISb 50. The company conducts a sensitivity analysis using a variable cost of $9. The totalvariable cost estimate will be:a. $21,375b. $22,500c. $23,625d. $24,125e. $24,750Difficulty level: MediumUse this information to answer questions 51 through 55.The Can-Do Co. is analyzing a proposed project. The company expects to sell 12,000units, give or take 4 percent. The expected variable cost per unit is $7 and the expectedfixed cost is $36,000. The fixed and variable cost estimates are considered accuratewithin a plus or minus 6 percent range. The depreciation expense is $30,000. The tax rate is 34 percent. The sale price is estimated at $14 a unit, give or take 5 percent. Thecompany bases their sensitivity analysis on the expected case scenario.SCENARIO ANALYSISa 51. What is the earnings before interest and taxes under the expected case scenario?a. $18,000b. $24,000c. $36,000d. $48,000e. $54,000Difficulty level: MediumSCENARIO ANALYSISc 52. What is the earnings before interest and taxes under anoptimistic case scenario?a. $22,694.40b. $24,854.40c. $37,497.60d. $52,694.40e. $67,947.60Difficulty level: ChallengeSCENARIO ANALYSISb 53. What is the earnings before interest and taxes under the pessimistic case scenario?b. -$422.40c. -$278.78d. $3,554.50e. $5,385.60Difficulty level: ChallengeSENSITIVITY ANALYSISd 54. What is the operating cash flow for a sensitivity analysis using total fixed costs of$32,000?a. $14,520b. $16,520c. $22,000d. $44,520e. $52,000Difficulty level: MediumSENSITIVITY ANALYSISd 55. What is the contribution margin for a sensitivity analysis using a variable cost per unitof $8?a. $3b. $4c. $5d. $6e. $7Difficulty level: MediumVARIABLE COSTc 56. A firm is reviewing a project with labor cost of $8.90 per unit, raw materials cost of$21.63 a unit, and fixed costs of $8,000 a month. Sales are projected at 10,000 unitsover the three-month life of the project. What are the total variable costs of the project?a. $216,300b. $297,300c. $305,300d. $313,300e. $329,300Difficulty level: MediumVARIABLE COSTd 57. A project has earnings before interest and taxes of $5,750, fixed costs of $50,000, aselling price of $13 a unit, and a sales quantity of 11,500 units. Depreciation is $7,500.What is the variable cost per unit?a. $6.75c. $7.25d. $7.50e. $7.75Difficulty level: MediumFIXED COSTb 58. At a production level of 5,600 units a project has total costs of $89,000. The variablecost per unit is $11.20. What is the amount of the total fixed costs?a. $24,126b. $26,280c. $27,090d. $27,820e. $28,626Difficulty level: MediumFIXED COSTe 59. At a production level of 6,000 units a project has total costs of $120,000. The variablecost per unit is $14.50. What is the amount of the total fixed costs?a. $25,165b. $28,200c. $30,570d. $32,000e. $33,000Difficulty level: MediumCONTRIBUTION MARGINc 60. Wilson’s Meats has computed their fixed costs to be $.60 for every pound of meatthey sell given an average daily sales level of 500 pounds. They charge $3.89 perpound of top-grade ground beef. The variable cost perpound is $2.99. What is thecontribution margin per pound of ground beef sold?a. $.30b. $.60c. $.90d. $2.99e. $3.89Difficulty level: MediumCONTRIBUTION MARGINe 61. Ralph and Emma’s is considering a project with total sales of $17,500, total variablecosts of $9,800, total fixed costs of $3,500, and estimated production of 400 units. Thedepreciation expense is $2,400 a year. What is the contribution margin per unit?a. $4.50b. $10.50d. $19.09e. $19.25Difficulty level: MediumACCOUNTING BREAK-EVENa 62. You are considering a new project. The project has projected depreciation of $720,fixed costs of $6,000, and total sales of $11,760. The variable cost per unit is$4.20. What is the accounting break-even level of production?a. 1,200 unitsb. 1,334 unitsc. 1,372 unitsd. 1,889 unitse. 1,910 unitsDifficulty level: MediumACCOUNTING BREAK-EVENb 63. The accounting break-even production quantity for a project is 5,425 units. The fixedcosts are $31,600 and the contribution margin is $6. What is the projecteddepreciation expense?a. $700b. $950c. $1,025d. $1,053e. $1,100Difficulty level: MediumACCOUNTING BREAK-EVENd 64. A project has an accounting break-even point of 2,000 units. The fixed costs are$4,200 and the depreciation expense is $400. The projected variable cost per unit is$23.10. What is the projected sales price?a. $20.80b. $21.00c. $21.20d. $25.40e. $25.60Difficulty level: MediumACCOUNTING BREAK-EVENa 65. A proposed project has fixed costs of $3,600,depreciation expense of $1,500, and asales quantity of 1,300 units. What is the contribution margin if the projected level ofsales is the accounting break-even point?a. $3.92c. $4.50d. $4.80e. $5.00Difficulty level: MediumPRESENT VALUE BREAK-EVENc 66. A project has a contribution margin of $5, projected fixed costs of $12,000, projectedvariable cost per unit of $12, and a projected present value break-even point of 5,000units. What is the operating cash flow at this level of output?a. $1,000b. $12,000c. $13,000d. $68,000e. $73,000Difficulty level: MediumPRESENT VALUE BREAK-EVENa 67. Thompson & Son have been busy analyzing a new product. They have determined thatan operating cash flow of $16,700 will result in a zero net present value, which is acompany requirement for project acceptance. The fixed costs are $12,378 and thecontribution margin is $6.20. The company feels that they can realistically capture10 percent of the 50,000 unit market for this product. Should the company develop thenew product? Why or why not?a. yes; because 5,000 units of sales exceeds the quantity required for a zero net presentvalueb. yes; because the internal break-even point is less than 5,000 unitsc. no; because the firm can not generate sufficient sales to obtain at least a zero netpresent valued. no; because the project has an expected internal rate of return of negative 100percente. no; because the project will not pay back on a discounted basisDifficulty level: ChallengePRESENT VALUE BREAK-EVENe 68. Kurt Neal and Son is considering a project with a discounted payback just equal to theproject’s life. The projections include a sales price of $11, variable cost per unit of$8.50, and fixed costs of $4,500. The operating cash flow is $6,200. What is the break-even quantity?a. 1,800 unitsb. 2,480 unitsc. 3,057 unitsd. 3,750 unitse. 4,280 unitsDECISION TREE NET PRESENT VALUEb 69. At stage 2 of the decision tree it shows that if a project is successful, the payoff will be$53,000 with a 2/3 chance of occurrence. There is also the 1/3 chance of a $-24,000payoff. The cost of getting to stage 2 (1 year out) is $44,000. The cost of capital is15%. What is the NPV of the project at stage 1?a. $-13,275b. $-20,232c. $ 2,087d. $ 7,536e. Can not be calculated without the exact timing of future cash flows.Difficulty level: MediumUse the following to answer questions 70-71:The Quick-Start Company has the following pattern of potential cash flows with their planned investment in a new cold weather starting system for fuel injected cars.DECISION TREEa 70. If the company has a discount rate of 17%, what is the value closest to time 1 netpresent value?a. $ 48.6 millionb. $ 80.9 millionc. $108.2 milliond. $181.4 millione. None of the above.DECISION TREEb 71. If the company has a discount rate of 17%, should they decide to invest?a. yes, NPV = $ 2.2 millionb. yes, NPV = $ 21.6 millionc. no, NPV = $-1.9 milliond. yes, NPV = $ 8.6 millione. No, since more than one branch is NPV = 0 or negative you must reject.Difficulty level: ChallengeACCOUNTING BREAK-EVENe 72. The Mini-Max Company has the following cost information on their new prospectiveproject. Calculate the accounting break-even point.Initial investment: $700。

公司理财第九版罗斯课后案例答案 Case Solutions Corporate Finance

公司理财第九版罗斯课后案例答案 Case Solutions CorporateFinance1. 案例一：公司资金需求分析问题：一家公司需要资金支持其新项目。

通过分析现金流量，推断该公司是否需要向外部借款或筹集其他资金。

解答：为了确定公司是否需要外部资金，我们需要分析公司的现金流量状况。

首先，我们需要计算公司的净现金流量（净收入加上非现金项目）。

然后，我们需要将净现金流量与项目的投资现金流量进行对比。

假设公司预计在项目开始时投资100万美元，并在项目运营期为5年。

预计该项目每年将产生50万美元的净现金流量。

现在，我们需要进行以下计算：净现金流量 = 年度现金流量 - 年度投资现金流量年度投资现金流量 = 100万美元年度现金流量 = 50万美元净现金流量 = 50万美元 - 100万美元 = -50万美元根据计算结果，公司的净现金流量为负数（即净现金流出），意味着公司每年都会亏损50万美元。

因此，公司需要从外部筹集资金以支持项目的运营。

2. 案例二：公司股权融资问题：一家公司正在考虑通过股权融资来筹集资金。

根据公司的财务数据和资本结构分析，我们需要确定公司最佳的股权融资方案。

解答：为了确定最佳的股权融资方案，我们需要参考公司的财务数据和资本结构分析。

首先，我们需要计算公司的资本结构比例，即股本占总资本的比例。

然后，我们将不同的股权融资方案与资本结构比例进行对比，选择最佳的方案。

假设公司当前的资本结构比例为60%的股本和40%的债务，在当前的资本结构下，公司的加权平均资本成本（WACC）为10%。

现在，我们需要进行以下计算：•方案一：以新股发行筹集1000万美元，并将其用于项目投资。

在这种方案下，公司的资本结构比例将发生变化。

假设公司的股本增加至80%，债务比例减少至20%。

根据资本结构比例的变化，WACC也将发生变化。

新的WACC可以通过以下公式计算得出：新的WACC = (股本比例 * 股本成本) + (债务比例 * 债务成本)假设公司的股本成本为12%，债务成本为8%：新的WACC = (0.8 * 12%) + (0.2 * 8%) = 9.6%•方案二：以新股发行筹集5000万美元，并将其用于项目投资。

罗斯《公司理财》第9版英文原书课后部分章节答案

罗斯《公司理财》第9版精要版英文原书课后部分章节答案详细»1 / 17 CH5 11，13，18，19，20 11. To find the PV of a lump sum, we use: PV = FV / (1 + r) t PV = $1,000,000 / (1.10) 80 = $488.19 13. To answer this question, we can use either the FV or the PV formula. Both will give the same answer since they are the inverse of each other. We will use the FV formula, that is: FV = PV(1 + r) t Solving for r, we get: r = (FV / PV) 1 / t –1 r = ($1,260,000 / $150) 1/112 – 1 = .0840 or 8.40% To find the FV of the first prize, we use: FV = PV(1 + r) t FV = $1,260,000(1.0840) 33 = $18,056,409.94 18. To find the FV of a lump sum, we use: FV = PV(1 + r) t FV = $4,000(1.11) 45 = $438,120.97 FV = $4,000(1.11) 35 = $154,299.40 Better start early! 19. We need to find the FV of a lump sum. However, the money will only be invested for six years, so the number of periods is six. FV = PV(1 + r) t FV = $20,000(1.084)6 = $32,449.33 20. To answer this question, we can use either the FV or the PV formula. Both will give the same answer since they are the inverse of each other. We will use the FV formula, that is: FV = PV(1 + r) t Solving for t, we get: t = ln(FV / PV) / ln(1 + r) t = ln($75,000 / $10,000) / ln(1.11) = 19.31 So, the money must be invested for 19.31 years. However, you will not receive the money for another two years. From now, you’ll wait: 2 years + 19.31 years = 21.31 years CH6 16，24，27，42，58 16. For this problem, we simply need to find the FV of a lump sum using the equation: FV = PV(1 + r) t 2 / 17 It is important to note that compounding occurs semiannually. To account for this, we will divide the interest rate by two (the number of compounding periods in a year), and multiply the number of periods by two. Doing so, we get: FV = $2,100[1 + (.084/2)] 34 = $8,505.93 24. This problem requires us to find the FV A. The equation to find the FV A is: FV A = C{[(1 + r) t – 1] / r} FV A = $300[{[1 + (.10/12) ] 360 – 1} / (.10/12)] = $678,146.38 27. The cash flows are annual and the compounding period is quarterly, so we need to calculate the EAR to make the interest rate comparable with the timing of the cash flows. Using the equation for the EAR, we get: EAR = [1 + (APR / m)] m – 1 EAR = [1 + (.11/4)] 4 – 1 = .1146 or 11.46% And now we use the EAR to find the PV of each cash flow as a lump sum and add them together: PV = $725 / 1.1146 + $980 / 1.1146 2 + $1,360 / 1.1146 4 = $2,320.36 42. The amount of principal paid on the loan is the PV of the monthly payments you make. So, the present value of the $1,150 monthly payments is: PV A = $1,150[(1 – {1 / [1 + (.0635/12)]} 360 ) / (.0635/12)] = $184,817.42 The monthly payments of $1,150 will amount to a principal payment of $184,817.42. The amount of principal you will still owe is: $240,000 – 184,817.42 = $55,182.58 This remaining principal amount will increase at the interest rate on the loan until the end of the loan period. So the balloon payment in 30 years, which is the FV of the remaining principal will be: Balloon payment = $55,182.58[1 + (.0635/12)] 360 = $368,936.54 58. To answer this question, we should find the PV of both options, and compare them. Since we are purchasing the car, the lowest PV is the best option. The PV of the leasing is simply the PV of the lease payments, plus the $99. The interest rate we would use for the leasing option is the same as the interest rate of the loan. The PV of leasing is: PV = $99 + $450{1 –[1 / (1 + .07/12) 12(3) ]} / (.07/12) = $14,672.91 The PV of purchasing the car is the current price of the car minus the PV of the resale price. The PV of the resale price is: PV = $23,000 / [1 + (.07/12)] 12(3) = $18,654.82 The PV of the decision to purchase is: $32,000 – 18,654.82 = $13,345.18 3 / 17 In this case, it is cheaper to buy the car than leasing it since the PV of the purchase cash flows is lower. To find the breakeven resale price, we need to find the resale price that makes the PV of the two options the same. In other words, the PV of the decision to buy should be: $32,000 – PV of resale price = $14,672.91 PV of resale price = $17,327.09 The resale price that would make the PV of the lease versus buy decision is the FV ofthis value, so: Breakeven resale price = $17,327.09[1 + (.07/12)] 12(3) = $21,363.01 CH7 3，18，21，22，31 3. The price of any bond is the PV of the interest payment, plus the PV of the par value. Notice this problem assumes an annual coupon. The price of the bond will be: P = $75({1 – [1/(1 + .0875)] 10 } / .0875) + $1,000[1 / (1 + .0875) 10 ] = $918.89 We would like to introduce shorthand notation here. Rather than write (or type, as the case may be) the entire equation for the PV of a lump sum, or the PV A equation, it is common to abbreviate the equations as: PVIF R,t = 1 / (1 + r) t which stands for Present V alue Interest Factor PVIFA R,t = ({1 – [1/(1 + r)] t } / r ) which stands for Present V alue Interest Factor of an Annuity These abbreviations are short hand notation for the equations in which the interest rate and the number of periods are substituted into the equation and solved. We will use this shorthand notation in remainder of the solutions key. 18. The bond price equation for this bond is: P 0 = $1,068 = $46(PVIFA R%,18 ) + $1,000(PVIF R%,18 ) Using a spreadsheet, financial calculator, or trial and error we find: R = 4.06% This is thesemiannual interest rate, so the YTM is: YTM = 2 4.06% = 8.12% The current yield is:Current yield = Annual coupon payment / Price = $92 / $1,068 = .0861 or 8.61% The effective annual yield is the same as the EAR, so using the EAR equation from the previous chapter: Effective annual yield = (1 + 0.0406) 2 – 1 = .0829 or 8.29% 20. Accrued interest is the coupon payment for the period times the fraction of the period that has passed since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six months is one-half of the annual coupon payment. There are four months until the next coupon payment, so two months have passed since the last coupon payment. The accrued interest for the bond is: Accrued interest = $74/2 × 2/6 = $12.33 And we calculate the clean price as: 4 / 17 Clean price = Dirty price –Accrued interest = $968 –12.33 = $955.67 21. Accrued interest is the coupon payment for the period times the fraction of the period that has passed since the last coupon payment. Since we have a semiannual coupon bond, the coupon payment per six months is one-half of the annual coupon payment. There are two months until the next coupon payment, so four months have passed since the last coupon payment. The accrued interest for the bond is: Accrued interest = $68/2 × 4/6 = $22.67 And we calculate the dirty price as: Dirty price = Clean price + Accrued interest = $1,073 + 22.67 = $1,095.67 22. To find the number of years to maturity for the bond, we need to find the price of the bond. Since we already have the coupon rate, we can use the bond price equation, and solve for the number of years to maturity. We are given the current yield of the bond, so we can calculate the price as: Current yield = .0755 = $80/P 0 P 0 = $80/.0755 = $1,059.60 Now that we have the price of the bond, the bond price equation is: P = $1,059.60 = $80[(1 – (1/1.072) t ) / .072 ] + $1,000/1.072 t We can solve this equation for t as follows: $1,059.60(1.072) t = $1,111.11(1.072) t –1,111.11 + 1,000 111.11 = 51.51(1.072) t2.1570 = 1.072 t t = log 2.1570 / log 1.072 = 11.06 11 years The bond has 11 years to maturity.31. The price of any bond (or financial instrument) is the PV of the future cash flows. Even though Bond M makes different coupons payments, to find the price of the bond, we just find the PV of the cash flows. The PV of the cash flows for Bond M is: P M = $1,100(PVIFA 3.5%,16 )(PVIF 3.5%,12 ) + $1,400(PVIFA3.5%,12 )(PVIF 3.5%,28 ) + $20,000(PVIF 3.5%,40 ) P M = $19,018.78 Notice that for the coupon payments of $1,400, we found the PV A for the coupon payments, and then discounted the lump sum back to today. Bond N is a zero coupon bond with a $20,000 par value, therefore, the price of the bond is the PV of the par, or: P N = $20,000(PVIF3.5%,40 ) = $5,051.45 CH8 4，18，20，22，244. Using the constant growth model, we find the price of the stock today is: P 0 = D 1 / (R – g) = $3.04 / (.11 – .038) = $42.22 5 / 17 18. The price of a share of preferred stock is the dividend payment divided by the required return. We know the dividend payment in Year 20, so we can find the price of the stock in Y ear 19, one year before the first dividend payment. Doing so, we get: P 19 = $20.00 / .064 P 19 = $312.50 The price of the stock today is the PV of the stock price in the future, so the price today will be: P 0 = $312.50 / (1.064) 19 P 0 = $96.15 20. We can use the two-stage dividend growth model for this problem, which is: P 0 = [D 0 (1 + g 1 )/(R – g 1 )]{1 – [(1 + g 1 )/(1 + R)] T }+ [(1 + g 1 )/(1 + R)] T [D 0 (1 + g 2 )/(R –g 2 )] P0 = [$1.25(1.28)/(.13 –.28)][1 –(1.28/1.13) 8 ] + [(1.28)/(1.13)] 8 [$1.25(1.06)/(.13 – .06)] P 0 = $69.55 22. We are asked to find the dividend yield and capital gains yield for each of the stocks. All of the stocks have a 15 percent required return, which is the sum of the dividend yield and the capital gains yield. To find the components of the total return, we need to find the stock price for each stock. Using this stock price and the dividend, we can calculate the dividend yield. The capital gains yield for the stock will be the total return (required return) minus the dividend yield. W: P 0 = D 0 (1 + g) / (R – g) = $4.50(1.10)/(.19 – .10) = $55.00 Dividend yield = D 1 /P 0 = $4.50(1.10)/$55.00 = .09 or 9% Capital gains yield = .19 – .09 = .10 or 10% X: P 0 = D 0 (1 + g) / (R – g) = $4.50/(.19 – 0) = $23.68 Dividend yield = D 1 /P 0 = $4.50/$23.68 = .19 or 19% Capital gains yield = .19 – .19 = 0% Y: P 0 = D 0 (1 + g) / (R – g) = $4.50(1 – .05)/(.19 + .05) = $17.81 Dividend yield = D 1 /P 0 = $4.50(0.95)/$17.81 = .24 or 24% Capital gains yield = .19 – .24 = –.05 or –5% Z: P 2 = D 2 (1 + g) / (R – g) = D 0 (1 + g 1 ) 2 (1 +g 2 )/(R – g 2 ) = $4.50(1.20) 2 (1.12)/(.19 – .12) = $103.68 P 0 = $4.50 (1.20) / (1.19) + $4.50(1.20) 2 / (1.19) 2 + $103.68 / (1.19) 2 = $82.33 Dividend yield = D 1 /P 0 = $4.50(1.20)/$82.33 = .066 or 6.6% Capital gains yield = .19 – .066 = .124 or 12.4% In all cases, the required return is 19%, but the return is distributed differently between current income and capital gains. High growth stocks have an appreciable capital gains component but a relatively small current income yield; conversely, mature, negative-growth stocks provide a high current income but also price depreciation over time. 24. Here we have a stock with supernormal growth, but the dividend growth changes every year for the first four years. We can find the price of the stock in Y ear 3 since the dividend growth rate is constant after the third dividend. The price of the stock in Y ear 3 will be the dividend in Y ear 4, divided by the required return minus the constant dividend growth rate. So, the price in Y ear 3 will be: 6 / 17 P3 = $2.45(1.20)(1.15)(1.10)(1.05) / (.11 – .05) = $65.08 The price of the stock today will be the PV of the first three dividends, plus the PV of the stock price in Y ear 3, so: P 0 = $2.45(1.20)/(1.11) + $2.45(1.20)(1.15)/1.11 2 + $2.45(1.20)(1.15)(1.10)/1.11 3 + $65.08/1.11 3 P 0 = $55.70 CH9 3，4，6，9，15 3. Project A has cash flows of $19,000 in Y ear 1, so the cash flows are short by $21,000 of recapturing the initial investment, so the payback for Project A is: Payback = 1 + ($21,000 / $25,000) = 1.84 years Project B has cash flows of: Cash flows = $14,000 + 17,000 + 24,000 = $55,000 during this first three years. The cash flows are still short by $5,000 of recapturing the initial investment, so the payback for Project B is: B: Payback = 3 + ($5,000 / $270,000) = 3.019 years Using the payback criterion and a cutoff of 3 years, accept project A and reject project B. 4. When we use discounted payback, we need to find the value of all cash flows today. The value today of the project cash flows for the first four years is: V alue today of Y ear 1 cash flow = $4,200/1.14 = $3,684.21 V alue today of Y ear 2 cash flow = $5,300/1.14 2 = $4,078.18 V alue today of Y ear 3 cash flow = $6,100/1.14 3 = $4,117.33 V alue today of Y ear 4 cash flow = $7,400/1.14 4 = $4,381.39 To findthe discounted payback, we use these values to find the payback period. The discounted first year cash flow is $3,684.21, so the discounted payback for a $7,000 initial cost is: Discounted payback = 1 + ($7,000 – 3,684.21)/$4,078.18 = 1.81 years For an initial cost of $10,000, the discounted payback is: Discounted payback = 2 + ($10,000 –3,684.21 –4,078.18)/$4,117.33 = 2.54 years Notice the calculation of discounted payback. We know the payback period is between two and three years, so we subtract the discounted values of the Y ear 1 and Y ear 2 cash flows from the initial cost. This is the numerator, which is the discounted amount we still need to make to recover our initial investment. We divide this amount by the discounted amount we will earn in Y ear 3 to get the fractional portion of the discounted payback. If the initial cost is $13,000, the discounted payback is: Discounted payback = 3 + ($13,000 – 3,684.21 – 4,078.18 – 4,117.33) / $4,381.39 = 3.26 years 7 / 17 6. Our definition of AAR is the average net income divided by the average book value. The average net income for this project is: A verage net income = ($1,938,200 + 2,201,600 + 1,876,000 + 1,329,500) / 4 = $1,836,325 And the average book value is: A verage book value = ($15,000,000 + 0) / 2 = $7,500,000 So, the AAR for this project is: AAR = A verage net income / A verage book value = $1,836,325 / $7,500,000 = .2448 or 24.48% 9. The NPV of a project is the PV of the outflows minus the PV of the inflows. Since the cash inflows are an annuity, the equation for the NPV of this project at an 8 percent required return is: NPV = –$138,000 + $28,500(PVIFA 8%, 9 ) = $40,036.31 At an 8 percent required return, the NPV is positive, so we would accept the project. The equation for the NPV of the project at a 20 percent required return is: NPV = –$138,000 + $28,500(PVIFA 20%, 9 ) = –$23,117.45 At a 20 percent required return, the NPV is negative, so we would reject the project. We would be indifferent to the project if the required return was equal to the IRR of the project, since at that required return the NPV is zero. The IRR of the project is: 0 = –$138,000 + $28,500(PVIFA IRR, 9 ) IRR = 14.59% 15. The profitability index is defined as the PV of the cash inflows divided by the PV of the cash outflows. The equation for the profitability index at a required return of 10 percent is: PI = [$7,300/1.1 + $6,900/1.1 2 + $5,700/1.1 3 ] / $14,000 = 1.187 The equation for the profitability index at a required return of 15 percent is: PI = [$7,300/1.15 + $6,900/1.15 2 + $5,700/1.15 3 ] / $14,000 = 1.094 The equation for the profitability index at a required return of 22 percent is: PI = [$7,300/1.22 + $6,900/1.22 2 + $5,700/1.22 3 ] / $14,000 = 0.983 8 / 17 We would accept the project if the required return were 10 percent or 15 percent since the PI is greater than one. We would reject the project if the required return were 22 percent since the PI。

公司理财-罗斯课后习题答案.doc

第一章1.在所有权形式的公司中,股东是公司的所有者。

股东选举公司的董事会,董事会任命该公司的管理层。

企业的所有权和控制权分离的组织形式是导致的代理关系存在的主要原因。

管理者可能追求自身或别人的利益最大化,而不是股东的利益最大化。

在这种环境下,他们可能因为目标不一致而存在代理问题。

2.非营利公司经常追求社会或政治任务等各种目标。

非营利公司财务管理的目标是获取并有效使用资金以最大限度地实现组织的社会使命。

3.这句话是不正确的。

管理者实施财务管理的目标就是最大化现有股票的每股价值，当前的股票价值反映了短期和长期的风险、时间以及未来现金流量。

4.有两种结论。

一种极端,在市场经济中所有的东西都被定价。

因此所有目标都有一个最优水平，包括避免不道德或非法的行为，股票价值最大化。

另一种极端,我们可以认为这是非经济现象,最好的处理方式是通过政治手段。

一个经典的思考问题给出了这种争论的答案:公司估计提高某种产品安全性的成本是30美元万。

然而,该公司认为提高产品的安全性只会节省20美元万。

请问公司应该怎么做呢?”5.财务管理的目标都是相同的,但实现目标的最好方式可能是不同的,因为不同的国家有不同的社会、政治环境和经济制度。

6.管理层的目标是最大化股东现有股票的每股价值。

如果管理层认为能提高公司利润，使股价超过35美元,那么他们应该展开对恶意收购的斗争。

如果管理层认为该投标人或其它未知的投标人将支付超过每股35美元的价格收购公司,那么他们也应该展开斗争。

然而,如果管理层不能增加企业的价值,并且没有其他更高的投标价格,那么管理层不是在为股东的最大化权益行事。

现在的管理层经常在公司面临这些恶意收购的情况时迷失自己的方向。

7.其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。

较少的私人投资者能减少不同的企业目标。

高比重的机构所有权导致高学历的股东和管理层讨论决策风险项目。

此外,机构投资者比私人投资者可以根据自己的资源和经验更好地对管理层实施有效的监督机制。

罗斯公司理财英文第九版Chap005

5-8
5.4 The Internal Rate of Return

IRR: the discount rate that sets NPV to zero Minimum Acceptance Criteria:

Ranking Criteria:

Accept if the IRR exceeds the required return Select alternative with the highest IRR

Independent Projects: accepting or rejecting one project does not affect the decision of the other projects.

Must exceed a MINIMUM acceptance criteria
5-2
5.1 Why Use Net Present Value?

Accepting positive NPV projects benefits shareholders.
NPV
uses cash flows NPV uses all the cash flows of the project NPV discounts the cash flows properly

Mutually Exclusive Projects: only ONE of several potential projects can be chosen, e.g., acquiring an accounting system.

RANK all alternatives, and select the best one.

英文版罗斯公司理财习题答案Chap001

CHAPTER 1INTRODUCTION TO CORPORATE FINANCEAnswers to Concept Questions1.The three basic forms are sole proprietorships, partnerships, and corporations. The advantages anddisadvantages of sole proprietorships and partnerships are: Disadvantages: unlimited liability, limited life, difficulty in transferring ownership, hard to raise capital funds. Some advantages: simpler, less regulation, the owners are also the managers, sometimes personal tax rates are better than corporate tax rates. The primary disadvantage of the corporate form is the double taxation to shareholders of distributed earnings and dividends. Some advantages include: limited liability, ease of transferability, ability to raise capital, and unlimited life. When a business is started, most take the form of a sole proprietorship or partnership.2.To maximize the current market value (share price) of the equity of the firm (whether it’s publiclytraded or not).3. In the corporate form of ownership, the shareholders are the owners of the firm. The shareholderselect the directors of the corporation, who in turn appoint the firm’s management. This separation of ownership from control in the corporate form of organization is what causes agency problems to exist. Management may act in its own or someone else’s best interests, rather than those of the shareholders. If such events occur, they may contradict the goal of maximizing the share price of the equity of the firm.4.Such organizations frequently pursue social or political missions, so many different goals areconceivable. One goal that is often cited is revenue minimization; i.e., provide whatever goods and services are offered at the lowest possible cost to society. A better approach might be to observe that even a not-for-profit business has equity. Thus, one answer is that the appropriate goal is to maximize the value of the equity.5.Presumably, the current stock value reflects the risk, timing, and magnitude of all future cash flows,both short-term and long-term. If this is correct, then the statement is false.6.An argument can be made either way. At the one extreme, we could argue that in a market economy,all of these things are priced. There is thus an optimal level of, for example, ethical and/or illegal behavior, and the framework of stock valuation explicitly includes these. At the other extreme, we could argue that these are non-economic phenomena and are best handled through the political process. A classic (and highly relevant) thought question that illustrates this debate goes something like this: “A firm has estimated that the cost of improving the safety of one of its products is $30 million. However, the firm believes that improving the safety of the product will only save $20 million in product liability claims. Wha t should the firm do?”7.The goal will be the same, but the best course of action toward that goal may be different because ofdiffering social, political, and economic institutions.8.The goal of management should be to maximize the share price for the current shareholders. Ifmanagement believes that it can improve the profitability of the firm so that the share price will exceed $35, then they should fight the offer from the outside company. If management believes that this bidder or other unidentified bidders will actually pay more than $35 per share to acquire the company, then they should still fight the offer. However, if the current management cannot increase the value of the firm beyond the bid price, and no other higher bids come in, then management is not acting in the interests of the shareholders by fighting the offer. Since current managers often lose their jobs when the corporation is acquired, poorly monitored managers have an incentive to fight corporate takeovers in situations such as this.9.We would expect agency problems to be less severe in other countries, primarily due to the relativelysmall percentage of individual ownership. Fewer individual owners should reduce the number of diverse opinions concerning corporate goals. The high percentage of institutional ownership might lead to a higher degree of agreement between owners and managers on decisions concerning risky projects. In addition, institutions may be better able to implement effective monitoring mechanisms on managers th an can individual owners, based on the institutions’ deeper resources and experiences with their own management. The increase in institutional ownership of stock in the United States and the growing activism of these large shareholder groups may lead to a reduction in agency problems for U.S. corporations and a more efficient market for corporate control.10. How much is too much? Who is worth more, Jack Welch or Tiger Woods? The simplest answer isthat there is a market for executives just as there is for all types of labor. Executive compensation is the price that clears the market. The same is true for athletes and performers. Having said that, one aspect of executive compensation deserves comment. A primary reason that executive compensation has grown so dramatically is that companies have increasingly moved to stock-based compensation.Such movement is obviously consistent with the attempt to better align stockholder and management interests. In recent years, stock prices have soared, so management has cleaned up. It is sometimes argued that much of this reward is simply due to rising stock prices in general, not managerial performance. Perhaps in the future, executive compensation will be designed to reward only differential performance, i.e., stock price increases in excess of general market increases.。

罗斯《公司理财》英文习题答案DOCchap

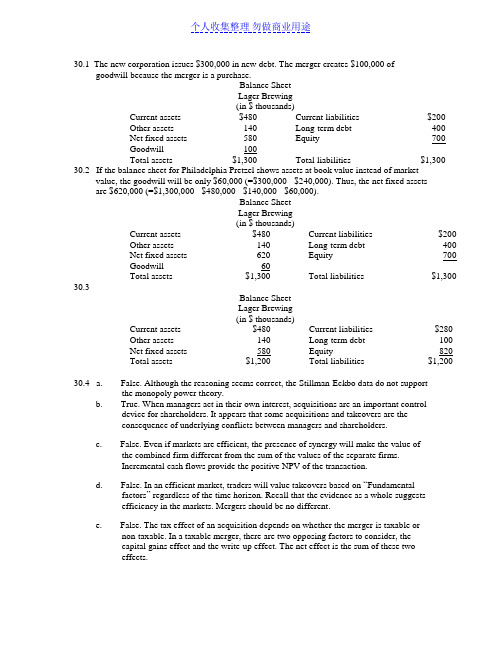
30.1 The new corporation issues $300,000 in new debt. The merger creates $100,000 ofgoodwill because the merger is a purchase.Balance SheetLager Brewing(in $ thousands)Current assets $480 Current liabilities $200Other assets 140 Long-term debt 400Net fixed assets 580 Equity 700Goodwill 100Total assets $1,300 Total liabilities $1,300 30.2 If the balance sheet for Philadelphia Pretzel shows assets at book value instead of marketvalue, the goodwill will be only $60,000 (=$300,000 - $240,000). Thus, the net fixed assetsare $620,000 (=$1,300,000 - $480,000 - $140,000 - $60,000).Balance SheetLager Brewing(in $ thousands)Current assets $480 Current liabilities $200Other assets 140 Long-term debt 400Net fixed assets 620 Equity 700Goodwill 60Total assets $1,300 Total liabilities $1,300 30.3Balance SheetLager Brewing(in $ thousands)Current assets $480 Current liabilities $280Other assets 140 Long-term debt 100Net fixed assets 580 Equity 820Total assets $1,200 Total liabilities $1,200 30.4 a. False. Although the reasoning seems correct, the Stillman-Eckbo data do not supportthe monopoly power theory.b. True. When managers act in their own interest, acquisitions are an important controldevice for shareholders. It appears that some acquisitions and takeovers are theconsequence of underlying conflicts between managers and shareholders.c. False. Even if markets are efficient, the presence of synergy will make the value ofthe combined firm different from the sum of the values of the separate firms.Incremental cash flows provide the positive NPV of the transaction.d. False. In an efficient market, traders will value takeovers based on “Fundamentalfactors” regardless of the time horizon. Recall that the evidence as a whole suggestsefficiency in the markets. Mergers should be no different.e. False. The tax effect of an acquisition depends on whether the merger is taxable ornon-taxable. In a taxable merger, there are two opposing factors to consider, thecapital gains effect and the write-up effect. The net effect is the sum of these twoeffects.f. True. Because of the coinsurance effect, wealth might be transferred from thestockholders to the bondholders. Acquisition analysis usually disregards this effectand considers only the total value.30.530.6 a. The weather conditions are independent. Thus, the joint probabilities are theproducts of the individual probabilities.Possible states Joint probabilityRain Rain 0.1 x 0.1=0.01Rain Warm 0.1 x 0.4=0.04Rain Hot 0.1 x 0.5=0.05Warm Rain 0.4 x 0.1=0.04Warm Warm 0.4 x 0.4=0.16Warm Hot 0.4 x 0.5=0.20Hot Rain 0.5 x 0.1=0.05Hot Warm 0.5 x 0.4=0.20Hot Hot 0.5 x 0.5=0.25Since the state Rain Warm has the same outcome (revenue) as Warm Rain, theirprobabilities can be added. The same is true of Rain Hot, Hot Rain and Warm Hot,Hot Warm. Thus the joint probabilities arePossibleJoint probabilitystatesRain Rain 0.01Rain Warm 0.08Rain Hot 0.10Warm Warm 0.16Warm Hot 0.40Hot Hot 0.25The joint values are the sums of the values of the two companies for the particularstate.Possible states Joint valueRain Rain $200,000Rain Warm 300,000Warm Warm 400,000Rain Hot 500,000Warm Hot 600,000Hot Hot 800,000b. Recall, if a firm cannot service its debt, the bondholders receive the value of the assets.Thus, the value of the debt is the value of the company if the face value of the debt isgreater than the value of the company. If the value of the company is greater than the value of the debt, the value of the debt is its face value. Here the value of the common stock is always the residual value of the firm over the value of the debt.Joint Prob. Joint Value Debt Value Stock Value0.01 $200,000 $200,000 $00.08 300,000 300,000 00.16 400,000 400,000 00.10 500,000 400,000 100,0000.40 600,000 400,000 200,0000.25 800,000 400,000 400,000c. To show that the value of the combined firm is the sum of the individual values, youmust show that the expected joint value is equal to the sum of the separate expected values.Expected joint value= 0.01($200,000) + 0.08($300,000) + 0.16($400,000) + 0.10($500,000) +0.40($600,000) + 0.25($800,000)= $580,000Since the firms are identical, the sum of the expected values is twice the expectedvalue of either.Expected individual value = 0.1($100,000) + 0.4($200,000) + 0.5($400,000) = $290,000 Expected combined value = 2($290,000) = $580,000d. The bondholders are better off if the value of the debt after the merger is greater thanthe value of the debt before the merger.Value of the debt before the merger:The value of debt for either company= 0.1($100,000) + 0.4($200,000) + 0.5($200,000) = $190,000Total value of debt before the merger = 2($190,000) = $380,000Value of debt after the merger= 0.01($200,000) + 0.08($300,000) + 0.16($400,000) + 0.10($400,000) +0.40($400,000) +0.25($400,000)= $390,000The bondholders are $10,000 better off after the merger.30.7 The decision hinges upon the risk of surviving. The final decision should hinge on thewealth transfer from bondholders to stockholders when risky projects are undertaken.High-risk projects will reduce the expected value of the bondholders’ claims on the firm.The telecommunications business is riskier than the utilities business. If the total value of the firm does not change, the increase in risk should favor the stockholder. Hence,management should approve this transaction. Note, if the total value of the firm dropsbecause of the transaction and the wealth effect is lower than the reduction in total value, management should reject the project.30.8 If the market is “smart,” the P/E ratio will not be constant.a. Value = $2,500 + $1,000 = $3,500b. EPS = Post-merger earnings / Total number of shares=($100 + $100)/200 =$1c. Price per share = Value/Total number of shares=$3,500/200 =$17.50d. If the market is “fooled,” the P/E ratio will be constant at $25.Value = P/E * Total number of shares= 25 * 200 = $5,000EPS = Post-merger earnings / Total number of shares=$5,000/200 = $25.0030.9 a. After the merger, Arcadia Financial will have 130,000 [=10,000 + (50,000)(6/10)]shares outstanding. The earnings of the combined firm will be $325,000. The earningsper share of the combined firm will be $2.50 (=$325,000/130,000). The acquisition will increase the EPS for the stockholders from $2.25 to $2.50.b. There will be no effect on the original Arcadia stockholders. No synergies exist in thismerger since Arcadia is buying Coldran at its market price. Examining the relativevalues of the two firms sees the latter point.Share price of Arcadia = (16 * $225,000) / 100,000=$36Share price of Coldran = (10.8 * $100,000) / 50,000=$21.60The relative value of these prices is $21.6/$36 = 0.6. Since Coldran’s shareholdersreceive 0.6 shares of Arcadia for every share of Coldran, no synergies exist.30.10 a. The synergy will be the discounted incremental cash flows. Since the cash flows areperpetual, this amount isb. The value of Flash-in-the-Pan to Fly-by-Night is the synergy plus the current marketvalue of Flash-in-the-Pan.V = $7,500,000 + $20,000,000= $27,500,000c. Cash alternative = $15,000,000Stock alternative = 0.25($27,500,000 + $35,000,000)= $15,625,000d. NPV of cash alternative = V - Cost=$27,500,000 - $15,000,000=$12,500,000NPV of stock alternative = V - Cost=$27,500,000 - $15,625,000=$11,875,000e. Use the cash alternative, its NPV is greater.30.11 a. The value of Portland Industries before the merger is $9,000,000 (=750,000x12). Thisvalue is also the discounted value of the expected future dividends.$9,000,000 =r = 0.1025 = 10.25%r is the risk-adjusted discount rate for Portland’s expected future dividends.the value of Portland Industries after the merger isThis is the value of Portland Industries to Freeport.b. NPV = Gain - Cost= $14,815,385 - ($40x250, 000)= $4,815,385c. If Freeport offers stock, the value of Portland Industries to Freeport is the same, but thecost differs.Cost = (Fraction of combined firm owned by Portland’s stockholders)x(Value of the combined firm)Value of the combined firm = (Value of Freeport before merger)+ (Value of Portland to Freeport)= $15x1,000,000 + $14,815,385= $29,815,385Cost = 0.375x$29,815,385= $11,180,769NPV= $14,815,385 - $11,180,769=$3,634,616d. The acquisition should be attempted with a cash offer since it provides a higher NPV.e. The value of Portland Industries after the merger isThis is the value of Portland Industries to Freeport.NPV = Gain-Cost=$11,223,529 - ($40x250,000)=$1,223,529If Freeport offers stock, the value of Portland Industries to Freeport is the same, but the cost differs.Cost = (Fraction of combined firm owned by Portland’s stockholders)x(Value of the combined firm)Value of the combined firm = (Value of Freeport before merger)+ (Value of Portland to Freeport)= $15x1,000,000 + $11,223,529= $26,223,529Cost = 0.375 * $26,223,529=$9,833,823NPV = $11,223,529 - $9,833,823=$1,389,706The acquisition should be attempted with a stock offer since it provides a higher NPV.30.12 a. Number of shares after acquisition=30 + 15 = 45 milStock price of Harrods after acquisition = 1,000/45=22.22 poundsb. Value of Selfridge stockholders after merger:α * 1,000 = 300α = 30%New shares issued = 12.86 mil12.86:20 = 0.643:1The proper exchange ratio should be 0.643 to make the stock offer’s value to Selfridgeequivalent to the cash offer.30.13 To evaluate this proposal, look at the present value of the incremental cash flows.Cash Flows to Company A(in $ million)Year 0 1 2 3 4 5Acquisition of B -550Dividends from B 150 32 5 20 30 45Tax-loss carryforwards 25 25Terminal value 600Total -400 32 30 45 30 645 The additional cash flows from the tax-loss carry forwards and the proposed level of debt should be discounted at the cost of debt because they are determined with very littleuncertainty.The after-tax cash flows are subject to normal business risk and must be discounted at anormal rate.Beta coefficient for the bond = 0.25 = [(8%-6%)/8%].Beta coefficient for the company = 1 = [(0.25)2 + (1.25)(0.75)]Discount rate for normal operations:r = 6% + 8% (1) = 14%Discount rate for dividends:The new beta coefficient for the company, 1, must be the weighted average of the debtbeta and the stock beta.1 = 0.5(0.25) + 0.5(βs)βs = 1.75r = 6% + 8%(1.75) = 20%Because the NPV of the acquisition is negative, Company A should not acquireCompany B.30.14 The commonly used defensive tactics by target-firm managers include:i. corporate charter amendments like super-majority amendment or staggering theelection of board members.ii. repurchase standstill agreements.iii. exclusionary self-tenders.iv. going private and leveraged buyouts.v. other devices like golden parachutes, scorched earth strategy, poison pill, ..., etc.Mini Case: U.S.Steel’s case.You have 3 choices: tender, or do not tender or sell in the market. If you do sell your shares in the market, at some point, somebody else would need to make a decision in “tender” or “not tender” as well.It is important to recognize that the firm has about 60 million shares outstanding (since 30 million shares will give US Steel 50.1% of Marathon shares). Let’s consider the possible sellingthe market price.If you choose not to tender, and 30 million shares were tendered US Steel succeeds to gain50.1% control, you will only receive $85 a share. If you do tender, the price you will receive will be no worse than $85 a share and can be as high as $125 a share. Depending on the number of shares tendered, you will receive one of the following prices.If only 50.1% tendered, you will get $125 per share.If the shares tendered exceed 50.1% but less than 100%, you will get more than $105 ashare.If all 60 million shares were tendered, you will get $105 per share. (which is )It is clear that, in the above 3 cases, when you are not sure about whether US Steel will succeed or not, you will be better off to tender your shares than not tender. This is because at best, you will only receive $85 per share if you choose not to tender.版权申明本文部分内容，包括文字、图片、以及设计等在网上搜集整理。

公司理财罗斯第9 版Chap005

Advantages:

5-10
IRR: Example
Consider the following project:
$50 $100 $150
0 -$200
1
2
3
The internal rate of return for this project is 19.44%
$50 $100 $150 NPV 0 200 2 (1 IRR ) (1 IRR ) (1 IRR ) 3

You first enter your range of cash flows, beginning with the initial cash flow. You can enter a guess, but it is not necessary. The default format is a whole percent – you will normally want to increase the decimal places to at least two.

5-5
5.2 The Payback Period Method
How long does it take the project to “pay back” its initial investment? Payback Period = number of years to recover initial costs Minimum Acceptance Criteria:
$150.00 $100.00 NPV $50.00 $0.00 -1% ($50.00) ($100.00) Discount rate
5-12

罗斯公司理财第六版习题答案第5章

Concept Questions◆Define pure discount bonds, level-coupon bonds, and consols.A pure discount bond is one that makes no intervening interest payments. One receives a single lump sum payment at maturity. A level-coupon bond is a combination of an annuity and a lump sum at maturity. A consol is a bond that makes interest payments forever.◆Contrast the state interest rate and the effective annual interest rate for bonds paying semi-annual interest. Effective annual interest rate on a bond takes into account two periods of compounding per year received on the coupon payments. The state rate does not take this into account.◆What is the relationship between interest rates and bond prices?There is an inverse relationship. When one goes up, the other goes down.◆How does one calculate the yield to maturity on a bond?One finds the discount rate that equates the promised future cash flows with the price of the bond.◆What are the three factors determining a firm's P/E ratio?Today's expectations of future growth opportunities.The discount rate.The accounting method.◆What is the closing price of General Data?The closing price of General Data is 6 3/16.◆What is the PE of General House?The PE of General House is 29.◆What is the annual dividend of General Host?The annual dividend of General Host is zero.Concept Questions- Appendix To Chapter 5◆What is the difference between a spot interest rate and the yield to maturity?The yield to maturity is the geometric average of the spot rates during the life of the bond.◆Define the forward rate.Given a one-year bond and a two-year bond, one knows the spot rates for both. The forward rate is the rate of return implicit on a one-year bond purchased in the second year that would equate the terminal wealth of purchasing the one-year bond today and another in one year with that of the two-year bond.◆What is the relationship between the one-year spot rate, the two-year spot rate and the forward rate over the second year?The forward rate f2 = [(1+r2)2 /(1+r1 )] - 1◆What is the expectation hypothesis?Investors set interest rates such that the forward rate over a given period equals the spot rate for that period.◆What is the liquidity-preference hypothesis?This hypothesis maintains that investors require a risk premium for holding longer-term bonds (i.e. they prefer to be liquid or short-term investors). This implies that the market sets the forward rate for a given period above the expected spot rate for that period.Questions And ProblemsHow to Value Bonds5.1 What is the present value of a 10-year, pure discount bond that pays $1,000 at maturity andis priced to yield the following rates?a. 5 percentb. 10 percentc. 15 percentSolutions a. $1,000 / 1.0510 = $613.91b. $1,000 / 1.1010 = $385.54c. $1,000 / 1.1510 = $247.185.2 Microhard has issued a bond with the following characteristics:Principal: $1,000Term to maturity: 20 yearsCoupon rate: 8 percentSemiannual paymentsCalculate the price of the Microhard bond if the stated annual interest rate is:a. 8 percentb. 10 percentc. 6 percentSolutions The amount of the semi-annual interest payment is $40 (=$1,000 ⨯ 0.08 / 2). There are a total of 40 periods; i.e., two half years in each of the twenty years in the term to maturity.The annuity factor tables can be used to price these bonds. The appropriate discount rate touse is the semi-annual rate. That rate is simply the annual rate divided by two. Thus, for part b the rate to be used is 5% and for part c is it 3%.a. $40 (19.7928) + $1,000 / 1.0440 = $1,000Notice that whenever the coupon rate and the market rate are the same, the bond ispriced at par.b. $40 (17.1591) + $1,000 / 1.0540 = $828.41Notice that whenever the coupon rate is below the market rate, the bond is pricedbelow par.c. $40 (23.1148) + $1,000 / 1.0340 = $1,231.15Notice that whenever the coupon rate is above the market rate, the bond is pricedabove par.5.3 Consider a bond with a face value of $1,000. The coupon is paid semiannually and themarket interest rate (effective annual interest rate) is 12 percent. How much would you payfor the bond ifa. the coupon rate is 8 percent and the remaining time to maturity is 20 years?b. the coupon rate is 10 percent and the remaining time to maturity is 15 years?Solutions Semi-annual discount factor = (1.12)1/2 - 1 = 0.05830 = 5.83%a. Price = $40400583.0A+ $1,000 / 1.058340= $614.98 + $103.67= $718.65b. Price = $50300583.0A+ $1,000 / 1.058330= $700.94 + $182.70 = $883.645.4 Pettit Trucking has issued an 8-percent, 20-year bond that pays interest semiannually. If themarket prices the bond to yield an effective annual rate of 10 percent, what is the price ofthe bond? Solutions Effective annual rate of 10%:Semi-annual discount factor = (1.1)0.5 - 1 = 0.04881 = 4.881%Price = $404004881.0A+ $1,000 / 1.0488140= $846.335.5 A bond is sold at $923.14 (below its par value of $1,000). The bond has 15 years tomaturity and investors require a 10-percent yield on the bond. What is the coupon rate forthe bond if the coupon is paid semiannually?Solutions $923.14 = C3005.0A+ $1,000 / 1.0530= (15.37245) C + $231.38C = $45The annual coupon rate = $45 ⨯ 2 / $1,000 = 0.09 = 9%5.6 You have just purchased a newly issued $1,000 five-year Vanguard Company bond at par.This five-year bond pays $60 in interest semiannually. You are also considering the purchaseof another Vanguard Company bond that returns $30 in semiannual interest payments andhas six years remaining before it matures. This bond has a face value of $1,000.a. What is effective annual return on the five-year bond?b. Assume that the rate you calculated in part (a) is the correct rate for the bond with sixyears remaining before it matures. What should you be willing to pay for that bond?c. How will your answer to part (b) change if the five-year bond pays $40 in semiannualinterest? Solutionsa. The semi-annual interest rate is $60 / $1,000 = 0.06. Thus, the effective annual rate is 1.062 - 1 =0.1236 = 12.36%.b. Price = $301206.0A+ $1,000 / 1.0612= $748.48c. Price = $301204.0A+ $1,000 / 1.0412= $906.15Note: In parts b and c we are implicitly assuming that the yield curve is flat. That is, the yield in year 5 applies for year 6 as well.Bond Concepts5.7 Consider two bonds, bond A and bond B, with equal rates of 10 percent and the same facevalues of $1,000. The coupons are paid annually for both bonds. Bond A has 20 years tomaturity while bond B has10 years to maturity.a. What are the prices of the two bonds if the relevant market interest rate is 10 percent?b. If the market interest rate increases to 12 percent, what will be the prices of the two bonds?c. If the market interest rate decreases to 8 percent, what will be the prices of the two bonds? Solutionsa. PA = $1002010.0A+ $1,000 / 1.1020 = $1,000PB = $1001010.0A+ $1,000 / 1.1010 = $1,000b. PA = $1002012.0A+ $1,000 / 1.1220 = $850.61PB = $1001012.0A+ $1,000 / 1.1210 = $887.00c. PA = $1002008.0A+ $1,000 / 1.0820 = $1,196.36PB = $1001008.0A+ $1,000 / 1.0810 = $1,134.205.8 a. If the market interest rate (the required rate of return that investors demand)unexpectedly increases, what effect would you expect this increase to have on theprices of long-term bonds? Why?b. What would be the effect of the rise in the interest rate on the general level of stockprices? Why? Solutionsa. The price of long-term bonds should fall. The price is the PV of the cash flowsassociated with the bond. As the interest rate rises, the PV of those flows falls.This can be easily seen by looking at a one-year, pure discount bond.Price = $1,000 / (1 + i)As i. increases, the denominator rises. This increase causes the price to fall.b. The effect upon stocks is not as certain as that upon the bonds. The nominalinterest rate is a function of both the real interest rate and the inflation rate; i.e.,(1 + i) = (1 + r) (1 + inflation)From this relationship it is easy to conclude that as inflation rises, the nominal interest rate rises. Stock prices are a function of dividends and future prices as well as the interest rate. Those dividends and future prices are determined by the earning power of the firm. When inflation occurs, it may increase or decrease firm earnings. Thus, the effect of a rise in the level of general prices upon the level of stock prices is uncertain.5.9 Consider a bond that pays an $80 coupon annually and has a face value of $1,000.Calculate the yield to maturity if the bond hasa. 20 years remaining to maturity and it is sold at $1,200.b. 10 years remaining to maturity and it is sold at $950.Solutions a. $1,200 = $8020rA+ $1,000 / (1 + r)20r = 0.0622 = 6.22%b. $950 = $8010rA+ $1,000 / (1 + r)10r = 0.0877 = 8.77%5.10 The Sue Fleming Corporation has two different bonds currently outstanding. Bond A has aface value of $40,000 and matures in 20 years. The bond makes no payments for the firstsix years and then pays $2,000 semiannually for the subsequent eight years, and finallypays $2,500 semiannually for the last six years. Bond B also has a face value of $40,000and a maturity of 20 years; it makes no coupon payments over the life of the bond. If therequired rate of return is 12 percent compounded semiannually, what is the current price ofBond A? of Bond B?Solutions PA = ($2,0001606.0A) / (1.06)12 + ($2,5001206.0A) / (1.06)28 + $40,000 / (1.06)40= $18,033.86PB = $ 40,000 / (1.06)40 = $3,888.89The Present Value of Common Stocks5.11 Use the following February 11, 2000, WSJ quotation for AT&T Corp. Which of thefollowing statements is false?a. The closing price of the bond with the shortest time to maturity was $1,000.b. The annual coupon for the bond maturing in year 2016 is $90.00.c. The price on the day before this quotation (i.e., February 9) for the ATT bond maturingin year 2022 was $1.075 per bond contract.d. The current yield on the ATT bond maturing in year 2002 was 7.125%e. The ATT bond maturing in year 2002 has a yield to maturity less than 7.125%.Bonds Cur Yld Vol Close Net ChgATT 9s 16 ? 10 117 _ 1/4ATT 5 1/8 01 ? 5 100 _ 3/4ATT 7 1/8 02 ? 193 104 1/8 _ 1/4ATT 8 1/8 22 ? 39 107 3/8 _ 1/8Solutions a. TrueTrueFalseFalseTrue5.12 Following are selected quotations for New York Exchange Bonds from the Wall StreetJournal. Which of the following statements about Wilson’s bond is false?a. The bond maturing in year 2000 has a yield to maturity greater than 63⁄8%.b. The closing price of the bond with the shortest time to maturity on the day before thisquotation was $1,003.25.c. This annual coupon for the bond maturing in year 2013 is $75.00.d. The current yield on the Wilson’s bond with the longest time to maturity was 7.29%.e. None of the above.Quotations as of 4 P.M. Eastern TimeFriday, April 23, 1999Bonds Current Yield Vol Close NetWILSON 6 3/8 99 ? 76 100 3/8 _ 1/8WILSON 6 3/8 00 ? 9 98 1/2WILSON 7 1/4 02 ? 39 103 5/8 1/8WILSON 7 1/2 13 ? 225 102 7/8 _ 1/8Solutions a. TrueFalseTrueTrueFalse5.13 A common stock pays a current dividend of $2. The dividend is expected to grow at an8-percent annual rate for the next three years; then it will grow at 4 percent in perpetuity.The appropriate discount rate is 12 percent. What is the price of this stock?Solutions Price = $2 (1.08) / 1.12 + $2 (1.082) / 1.122 + $2 (1.083) / 1.123+ {$2 (1.083) (1.04) / (0.12 - 0.04)} / 1.123= $28.895.14 Use the following February 12, 1998, WSJ quotation for Merck & Co. to answer the nextquestion. 52 Weeks Yld Vol NetHi Lo Stock Sym Div % PE 100s Hi Lo Close Chg120. 80.19 Merck MRK 1.80 ? 30 195111 115.9 114.5 115 _1.25Which of the following statements is false?a. The dividend yield was about 1.6%.b. The 52 weeks’ trading range was $39.81.c. The closing price per share on February 10, 1998, was $113.75.d. The closing price per share on February 11, 1998, was $115.e. The earnings per share were about $3.83.Solutions a. FalseTrueFalseFalseTrue5.15 Use the following stock quote.52 Weeks Yld Vol NetHi Lo Stock Sym Div % PE 100s Hi Lo Close Chg126.25 72.50 Citigroup CCI 1.30 1.32 16 20925 98.4 97.8 98.13 _.13The expected growth rate in Citigroup’s dividends is 7% a year. Suppose you use thediscounted dividend model to price Citigroup’s shares. The constant growth dividendmodel would suggest that the required return on the Citigroup’s stock is what?98.125 = 1.30 ( 1.07) / r - 0.07r = 8.4175 %5.16 You own $100,000 worth of Smart Money stock. At the end of the first year you receive adividend of $2 per share; at the end of year 2 you receive a $4 dividend. At the end of year3 you sell the stock for $50 per share. Only ordinary (dividend) income is taxed at the rateof 28 percent. Taxes are paid at the time dividends are received. The required rate of returnis 15 percent. How many shares of stock do you own? Solutions Price = $2 (0.72) / 1.15 + $4 (0.72) / 1.152 + $50 / 1.153= $36.31The number of shares you own = $100,000 / $36.31 = 2,754 shares5.17 Consider the stock of Davidson Company that will pay an annual dividend of $2 in thecoming year. The dividend is expected to grow at a constant rate of 5 percent permanently.The market requires a 12-percent return on the company.a. What is the current price of a share of the stock?b. What will the stock price be 10 years from today?Solutionsa. P = $2 / (0.12 - 0.05) = $28.57b. P10 = D11 / (r - g)= $2 (1.0510) / (0.12 - 0.05) = $46.545.18 Easy Type, Inc., is one of a myriad of companies selling word processor programs.Their newest program will cost $5 million to develop. First-year net cash flows will be$2 million. As a result of competition, profits will fall by 2 percent each year thereafter.All cash inflows will occur at year-end. If the market discount rate is 14 percent, what isthe value of this new program?SolutionsValue = -$5,000,000 + $2,000,000 / {0.14 - (-0.02)}= $7,500,0005.19 Whizzkids, Inc., is experiencing a period of rapid growth. Earnings and dividends pershare are expected to grow at a rate of 18 percent during the next two years, 15 percent inthe third year, and at a constant rate of 6 percent thereafter. Whizzkids’ last dividend,which has just been paid, was $1.15. If the required rate of return on the stock is 12percent, what is the price of a share of the stock today?SolutionsPrice = $1.15 (1.18) / 1.12 + $1.15 (1.182) / 1.122 + $1.152 (1.182) / 1.123+ {$1.152 (1.182) (1.06) / (0.12 - 0.06)} / 1.123= $26.955.20 Allen, Inc., is expected to pay an equal amount of dividends at the end of the first twoyears. Thereafter, the dividend will grow at a constant rate of 4 percent indefinitely. Thestock is currently traded at $30. What is the expected dividend per share for the next yearif the required rate of return is 12 percent?Solutions$30 = D / 1.12 + D / 1.122 + {D (1 + 0.04) / (0.12 - 0.04)} / 1.122= 12.053571 DD = $2.495.21 Calamity Mining Company’s reserves of ore are being depleted, and its costs ofrecovering a declining quantity of ore are rising each year. As a result, the company’searnings are declining at the rate of 10 percent per year. If the dividend per share that isabout to be paid is $5 and the required rate of return is 14 percent, what is the value of thefirm’s stock?SolutionsDividend one year from now = $5 (1 - 0.10) = $4.50Price = $5 + $4.50 / {0.14 - (-0.10)} = $23.75Since the current $5 dividend has not yet been paid, it is still included in the stock price.5.22 The Highest Potential, Inc., will pay a quarterly dividend per share of $1 at the end of eachof the next 12 quarters. Subsequently, the dividend will grow at a quarterly rate of 0.5percent indefinitely. The appropriate rate of return on the stock is 10 percent. What is thecurrent stock price?Estimates of Parameters in the Dividend-Discount ModelSolutionsPrice = $112025.0A+ {$1 (1 + 0.005) / (0.025 - 0.005)} / 1.02512= $10.26 + $37.36= $47.625.23 The newspaper reported last week that Bradley Enterprises earned $20 million. The reportalso stated that the firm’s return on equity remains on its historical trend of 14 percent.Bradley retains 60 percent of its earnings. What is the firm’s growth rate of earnings?What will next year’s earnings be?SolutionsGrowth rate g = 0.6 ⨯ 0.14 = 0.084 = 8.4%Next year earnings = $20 million ⨯ 1.084 = $21.68 million5.24 Von Neumann Enterprises has just reported earnings of $10 million, and it plans to retain 75percent of its earnings. The company has 1.25 million shares of common stock outstanding.The stock is selling at $30. The historical return on equity (ROE) of 12 percent is expectedto continue in the future. What is the required rate of return on the stock?Growth Opportunitiesg = retention ratio ⨯ ROE = 0.75 ⨯ 0.12= 0.09 = 9%Dividend per share = $10 million ⨯ (1 - 0.75) / 1.25 million= $2The required rate of return = $2 (1.09) / $30 + 0.09= 0.1627 = 16.27%5.25 Rite Bite Enterprises sells toothpicks. Gross revenues last year were $3 million, and totalcosts were $1.5 million. Rite Bite has 1 million shares of common stock outstanding.Gross revenues and costs are expected to grow at 5 percent per year. Rite Bite pays noincome taxes, and all earnings are paid out as dividends.a. If the appropriate discount rate is 15 percent and all cash flows are received at year’send, what is the price per share of Rite Bite stock?b. The president of Rite Bite decided to begin a program to produce toothbrushes. Theproject requires an immediate outlay of $15 million. In one year, another outlay of$5 million will be needed. The year after that, net cash inflows will be $6 million. Thisprofit level will be maintained in perpetuity. What effect will undertaking this projecthave on the price per share of the stock?Solutionsa. Price = ($3 - $1.5) ⨯ 1.05 / (0.15 - 0.05)= $15.75b. NPVGO = -$15,000,000 - $5,000,000 / 1.15 + ($6,000,000 / 0.15) / 1.15= $15,434,783The price increases by $15.43 per share.5.26 California Electronics, Inc., expects to earn $100 million per year in perpetuity if it doesnot undertake any new projects. The firm has an opportunity that requires an investment of$15 million today and $5 million in one year. The new investment will begin to generateadditional annual earnings of $10 million two years from today in perpetuity. The firm has20 million shares of common stock outstanding, and the required rate of return on thestock is 15 percent.a. What is the price of a share of the stock if the firm does not undertake the new project?b. What is the value of the growth opportunities resulting from the new project?c. What is the price of a share of the stock if the firm undertakes the new project?Solutionsa. Price = EPS / r = {$100 million / 20 million} / 0.15= $33.33b. NPV = -$15 million - $5 million / 1.15 + ($10 million / 0.15) / 1.15= $38,623,188c. Price = $33.33 + $38,623,188 / 20,000,000= $35.265.27 Suppose Smithfield Foods, Inc., has just paid a dividend of $1.40 per share. Sales andprofits for Smithfield Foods are expected to grow at a rate of 5% per year. Its dividend isexpected to grow by the same rate. If the required return is 10%, what is the value of ashare of Smithfield Foods?SolutionsPrice = 1.40 (1.05) / 0.10 - 0.05Price = $29.405.28 In order to buy back its own shares, Pennzoil Co. has decided to suspend its dividends forthe next two years. It will resume its annual cash dividend of $2.00 a share 3 years fromnow. This level of dividendswill be maintained for one more year. Thereafter, Pennzoil isexpected to increase its cash dividend payments by an annual growth rate of 6% per yearforever. The required rate of return on Pennzoil’s stock is 16%. According to thediscounted dividend model, what should Pennzoil’s current share price be? SolutionsPrice = 2 / (1.16) 3 + 2 / (1.16)4 + 2.12 / 0.16 - 0.06= 1.28 + 1.10 + 21.20= $23.585.29 Four years ago, Ultramar Diamond Inc. paid a dividend of $0.80 per share. This yearUltramar paid a dividend of $1.66 per share. It is expected that the company will paydividends growing at the same rate for the next 5 years. Thereafter, the growth rate willlevel at 8% per year. The required return on this stock is 18%. According to the discounteddividend model, what would Ultramar’s cash dividend be in 7 years?a. $2.86c. $3.68d. $4.30e. $4.82Solutionsa. g = 0.4 ⨯ 0.15 = 0.06 = 6%b. Dividend per share = $1.5 million ⨯ 0.6 / 300,000= $3Price = $3 (1.06) / (0.13 - 0.06)= $45.43c. Assuming the additional earnings generated are all paid out as cash dividends.NPV = -$1.2 million + $0.3 million {1 / (0.13 - 0.10)} {1 - (1.10 / 1.13)10}= $1,159,136.93d. Price = $45.43 + $1,159,136.93 / 300,000= $49.295.30 The Webster Co. has just paid a dividend of $5.25 per share. The company will increase itsdividend by 15 percent next year and will then reduce its dividend growth by 3 percenteach year until it reaches the industry average of 5 percent growth, after which thecompany will keep a constant growth, forever. The required rate of return for the WebsterCo. is 14 percent. What will a share of stock sell for?SolutionsPrice = 3 / 1.15 + 4.5 / ( 1.15)2 + 4.725 / 0.15- 0.05= 2.61 + 3.40 + 47.52= $53.535.31 Consider Pacific Energy Company and U.S. Bluechips, Inc., both of which reported recentearnings of $800,000 and have 500,000 shares of common stock outstanding. Assume bothfirms have the same required rate of return of 15 percent a year.a. Pacific Energy Company has a new project that will generate cash flows of $100,000each year in perpetuity. Calculate the P/E ratio of the company.Chapter 5 How to Value Bonds and Stocks 129b. U.S. Bluechips has a new project that will increase earnings by $200,000 in the comingyear. The increased earnings will grow at 10 percent a year in perpetuity. Calculate theP/E ratio of the firm. Solutionsa. P/E of Pacific Energy Company:EPS = ($800,000 / 500,000) = $1.6NPVGO = {$100,000 / 500,000} / 0.15 = $1.33P/E = 1 / 0.15 + 1.33 / 1.6 = 7.50b. P/E of U. S. Bluechips, Inc.:NPVGO = {$200,000 / 500,000} / (0.15 - 0.10) = $8P/E = 1 / 0.15 + 8 / 1.6 = 11.675.32 (Challenge Question) Lewin Skis Inc. (today) expects to earn $4.00 per share for each ofthe future operating periods (beginning at time 1) if the firm makes no new investments(and returns the earnings as dividends to the shareholders). However, Clint Williams,President and CEO, has discovered an opportunity to retain (and invest) 25% of theearnings beginning three years from today (starting at time 3). This opportunity to investwill continue (for each period) indefinitely. He expects to earn 40% (per year) on this newequity investment (ROE of 40), the return beginning one year after each investment ismade. The firm’s equity discount rate is 14% throughout.a. What is the price per share (now at time 0) of Lewin Skis Inc. stock without making thenew investment?b. If the new investment is expected to be made, per the preceding information, whatwould the value of the stock (per share) be now (at time 0)?c. What is the expected capital gain yield for the second period, assuming the proposedinvestment is made? What is the expected capital gain yield for the second period if theproposed investment is not made?d. What is the expected dividend yield for the second period if the new investment ismade? What is the expected dividend yield for the second period if the new investmentis not made?Solutionsa. Price = $4 / 0.14 = $28.57Price = 28.57 + (-1 + 0.40 / 0.14) / 0.04(1.14) 3= 28.57 + 31.33The expected return of 14% less the dividend yield of 5% providesa capital gain yield of 9%. If there is no investment the yield is 14%.$3 / $59.90 = .05 and $4 / $28.57 = .14 without the investment.Appendix to Chapter 5Questions And ProblemsA.1 The appropriate discount rate for cash flows received one year from today is 10 percent. Theappropriate annual discount rate for cash flows received two years from today is 11 percent.a. What is the price of a two-year bond that pays an annual coupon of 6 percent?b. What is the yield to maturity of this bond?Solutionsa. P = $60 / 1.10 + $1,060 / (1.11)2= $54.55 + $ 860.32= $914.87$914.87 = $60 / ( 1 + y ) + $1,060 / ( 1 + y )2y = YTM = 10.97%A.2 The one-year spot rate equals 10 percent and the two-year spot rate equals 8 percent. Whatshould a 5-percent coupon two-year bond cost?SolutionsP = $50 / 1.10 + $1,050 / (1.08)2= $45.45 + $900.21= $945.66A.3 If the one-year spot rate is 9 percent and the two-year spot rate is 10 percent, what is theforward rate? Solutions ( 1 + r1 )( 1 + ƒ2 ) = ( 1 + r2 )2( 1.09 ) ( 1 + ƒ2 ) = ( 1.10 )2ƒ2 = .1101A.4 Assume the following spot rates:Maturity Spot Rates (%)1 52 73 10What are the forward rates over each of the three years?Solutions( 1 + r2 )2 = ( 1+ r1 ) ( 1 + ƒ2 )( 1.07 )2 = ( 1.05 )( 1 + ƒ2 )ƒ2 = .0904, one-year forward rate over the 2nd year is 9.04%.( 1 + r3 )3 = ( 1 + r2 )2 ( 1 + ƒ3 )( 1.10 )3 = ( 1.07 )2 ( 1 + ƒ3 )ƒ3 = .1625, one-year forward rate over the 3rd year is 16.25%.。

(完整版)公司理财-罗斯课后习题答案

(完整版)公司理财-罗斯课后习题答案-CAL-FENGHAI-(2020YEAR-YICAI)_JINGBIAN第一章1.在所有权形式的公司中,股东是公司的所有者。

股东选举公司的董事会,董事会任命该公司的管理层。

企业的所有权和控制权分离的组织形式是导致的代理关系存在的主要原因。

管理者可能追求自身或别人的利益最大化,而不是股东的利益最大化。

在这种环境下,他们可能因为目标不一致而存在代理问题。

2.非营利公司经常追求社会或政治任务等各种目标。

非营利公司财务管理的目标是获取并有效使用资金以最大限度地实现组织的社会使命。

3.这句话是不正确的。

管理者实施财务管理的目标就是最大化现有股票的每股价值，当前的股票价值反映了短期和长期的风险、时间以及未来现金流量。

4.有两种结论。

一种极端,在市场经济中所有的东西都被定价。

因此所有目标都有一个最优水平，包括避免不道德或非法的行为，股票价值最大化。

另一种极端,我们可以认为这是非经济现象,最好的处理方式是通过政治手段。

一个经典的思考问题给出了这种争论的答案:公司估计提高某种产品安全性的成本是30美元万。

然而,该公司认为提高产品的安全性只会节省20美元万。

请问公司应该怎么做呢?”5.财务管理的目标都是相同的,但实现目标的最好方式可能是不同的,因为不同的国家有不同的社会、政治环境和经济制度。

6.管理层的目标是最大化股东现有股票的每股价值。

如果管理层认为能提高公司利润，使股价超过35美元,那么他们应该展开对恶意收购的斗争。

如果管理层认为该投标人或其它未知的投标人将支付超过每股35美元的价格收购公司,那么他们也应该展开斗争。

然而,如果管理层不能增加企业的价值,并且没有其他更高的投标价格,那么管理层不是在为股东的最大化权益行事。

现在的管理层经常在公司面临这些恶意收购的情况时迷失自己的方向。

7.其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。

较少的私人投资者能减少不同的企业目标。

英文版罗斯公司理财习题答案

CHAPTER 7NET PRESENT VALUE AND OTHER INVESTMENT CRITERIAAnswers to Concepts Review and Critical Thinking Questions1. A payback period less than the project’s life means that the NPV is positive for a zero discount rate,but nothing more definitive can be said. For discount rates greater than zero, the payback period will still be less than the project’s life, but the NPV may be positive, zero, or negative, depending on whether the discount rate is less than, equal to, or greater than the IRR. The discounted payback includes the effect of the relevant discount rate. If a project’s discounted payback period is less than the project’s life, it must be the case that NPV is positive.2.If a project has a positive NPV for a certain discount rate, then it will also have a positive NPV for azero discount rate; thus, the payback period must be less than the project life. Since discounted payback is calculated at the same discount rate as is NPV, if NPV is positive, the discounted payback period must be less than the project’s life. If NPV is positive, then the present value of future cash inflows is greater than the initial investment cost; thus PI must be greater than 1. If NPV is positive for a certain discount rate R, then it will be zero for some larger discount rate R*; thus, the IRR must be greater than the required return.3. a.Payback period is simply the accounting break-even point of a series of cash flows. To actuallycompute the payback period, it is assumed that any cash flow occurring during a given period isrealized continuously throughout the period, and not at a single point in time. The payback isthen the point in time for the series of cash flows when the initial cash outlays are fullyrecovered. Given some predetermined cutoff for the payback period, the decision rule is toaccept projects that payback before this cutoff, and reject projects that take longer to payback.The worst problem associated with payback period is that it ignores the time value of money. Inaddition, the selection of a hurdle point for payback period is an arbitrary exercise that lacksany steadfast rule or method. The payback period is biased towards short-term projects; it fullyignores any cash flows that occur after the cutoff point.b.The average accounting return is interpreted as an average measure of the accountingperformance of a project over time, computed as some average profit measure attributable tothe project divided by some average balance sheet value for the project. This text computesAAR as average net income with respect to average (total) book value. Given somepredetermined cutoff for AAR, the decision rule is to accept projects with an AAR in excess ofthe target measure, and reject all other projects. AAR is not a measure of cash flows and marketvalue, but a measure of financial statement accounts that often bear little resemblance to therelevant value of a project. In addition, the selection of a cutoff is arbitrary, and the time valueof money is ignored. For a financial manager, both the reliance on accounting numbers ratherthan relevant market data and the exclusion of time value of money considerations are troubling.Despite these problems, AAR continues to be used in practice because (1) the accountinginformation is usually available, (2) analysts often use accounting ratios to analyze firmperformance, and (3) managerial compensation is often tied to the attainment of targetaccounting ratio goals.c.The IRR is the discount rate that causes the NPV of a series of cash flows to be identically zero.IRR can thus be interpreted as a financial break-even rate of return; at the IRR discount rate,the net value of the project is zero. The acceptance and rejection criteria are:If C0 < 0 and all future cash flows are positive, accept the project if the internal rate ofreturn is greater than or equal to the discount rate.If C0 < 0 and all future cash flows are positive, reject the project if the internal rate ofreturn is less than the discount rate.If C0 > 0 and all future cash flows are negative, accept the project if the internal rate ofreturn is less than or equal to the discount rate.If C0 > 0 and all future cash flows are negative, reject the project if the internal rate ofreturn is greater than the discount rate.IRR is the interest rate that causes NPV for a series of cash flows to be zero. NPV is preferred in all situations to IRR; IRR can lead to ambiguous results if there are non-conventional cash flows, and it also ambiguously ranks some mutually exclusive projects. However, for stand-alone projects with conventional cash flows, IRR and NPV are interchangeable techniques. The IRR decision rule for projectsd.The profitability index is the present value of cash inflows relative to the project cost. As such,it is a benefit/cost ratio, providing a measure of the relative profitability of a project. The profitability index decision rule is to accept projects with a PI greater than one, and to reject projects with a PI less than one. The profitability index can be expressed as: PI = (NPV + cost)/cost = 1 + (NPV/cost). If a firm has a basket of positive NPV projects and is subject to capital rationing, PI may provide a good ranking measure of the projects, indicating the “bang for the buck” of each particu lar project.e.NPV is simply the present value of a project’s cash flows. NPV specifically measures, afterconsidering the time value of money, the net increase or decrease in firm wealth due to the project. The decision rule is to accept projects that have a positive NPV, and reject projects with a negative NPV. NPV is superior to the other methods of analysis presented in the text because it has no serious flaws. The method unambiguously ranks mutually exclusive projects, and can differentiate between projects of different scale and time horizon. The only drawback to NPV is that it relies on cash flow and discount rate values that are often estimates and not certain, but this is a problem shared by the other performance criteria as well. A project with NPV = $2,500 implies that the total shareholder wealth of the firm will increase by $2,500 if the project is accepted.4.For a project with future cash flows that are an annuity:Payback = I / CAnd the IRR is:0 = – I + C / IRRSolving the IRR equation for IRR, we get:IRR = C / INotice this is just the reciprocal of the payback. So:IRR = 1 / PBFor long-lived projects with relatively constant cash flows, the sooner the project pays back, the greater is the IRR.5.There are a number of reasons. Two of the most important have to do with transportation costs andexchange rates. Manufacturing in the U.S. places the finished product much closer to the point of sale, resulting in significant savings in transportation costs. It also reduces inventories because goods spend less time in transit. Higher labor costs tend to offset these savings to some degree, at least compared to other possible manufacturing locations. Of great importance is the fact that manufacturing in the U.S. means that a much higher proportion of the costs are paid in dollars. Since sales are in dollars, the net effect is to immunize profits to a large extent against fluctuations in exchange rates. This issue is discussed in greater detail in the chapter on international finance.6.The single biggest difficulty, by far, is coming up with reliable cash flow estimates. Determining anappropriate discount rate is also not a simple task. These issues are discussed in greater depth in the next several chapters. The payback approach is probably the simplest, followed by the AAR, but even these require revenue and cost projections. The discounted cash flow measures (discounted payback, NPV, IRR, and profitability index) are really only slightly more difficult in practice.7.Yes, they are. Such entities generally need to allocate available capital efficiently, just as for-profitsdo. However, it is frequently the case that the “revenues” from not-for-profit ventures are not tangible. For example, charitable giving has real opportunity costs, but the benefits are generally hard to measure. To the extent that benefits are measurable, the question of an appropriate required return remains. Payback rules are commonly used in such cases. Finally, realistic cost/benefit analysis along the lines indicated should definitely be used by the U.S. government and would go a long way toward balancing the budget!8.The statement is false. If the cash flows of Project B occur early and the cash flows of Project Aoccur late, then for a low discount rate the NPV of A can exceed the NPV of B. Observe the following example.C0C1C2IRR NPV @ 0% Project A –$1,000,000 $0 $1,440,000 20% $440,000 Project B –$2,000,000 $2,400,000 $0 20% 400,000However, in one particular case, the statement is true for equally risky Projects. If the lives of the two Projects are equal and the cash flows of Project B are twice the cash flows of Project A in every time period, the NPV of Project B will be twice the NPV of Project A.9. Although the profitability index (PI) is higher for Project B than for Project A, Project A should bechosen because it has the greater NPV. Confusion arises because Project B requires a smaller investment than Project A requires. Since the denominator of the PI ratio is lower for Project B than for Project A, B can have a higher PI yet have a lower NPV. Only in the case of capital rationing could the company’s decision have been incorrect.10. a.Project A would have a higher IRR since initial investment for Project A is less than that ofProject B, if the cash flows for the two projects are identical.b.Yes, since both the cash flows as well as the initial investment are twice that of Project B.11.Project B would have a more sensitive NPV to changes in the discount rate. The reason is the timevalue of money. Cash flows that occur further out in the future are always more sensitive to changes in the interest rate. This is similar to the interest rate risk of a bond.12.The MIRR is calculated by finding the present value of all cash outflows, the future value of all cashinflows to the end of the project, and then calculating the IRR of the two cash flows. As a result, the cash flows have been discounted or compounded by one interest rate (the required return), and then the interest rate between the two remaining cash flows is calculated. As such, the MIRR is not a true interest rate. In contrast, consider the IRR. If you take the initial investment, and calculate the future value at the IRR, you can replicate the future cash flows of the project exactly.13.The criticism is incorrect. It is true that if you calculate the future value of all intermediate cashflows to the end of the project at the required return, then calculate the NPV of this future value and the initial investment, you will get the same NPV. However, NPV says nothing about reinvestment of intermediate cash flows. The NPV is the present value of the project cash flows. The fact that the reinvestment works is an artifact of the time value of money.14.The criticism is incorrect for several reasons. It is true that if you calculate the future value of allintermediate cash flows to the end of the project at the IRR, then calculate the IRR of this future value and the initial investment, you will get the same IRR. This only occurs if the intermediate cash flows are reinvested at the IRR. However, similar to the previous question, IRR deals with the present value of the cash flows, not the future value. There is also another important point. This criticism deals with the reinvestment of the intermediate cash flows. As we will see in the next chapter, any reinvestment assumption concerning the intermediate cash flows is incorrect. The reason is that when we are calculating the cash flows for a project, we are concerned with the incremental cash flows from the project, that is, the cash flows the project creates. Reinvestment violates this principal. Consider the following example:C0C1C2IRR Project A –$100 $10 $110 10% Suppose this is a deposit into a bank account. The IRR of the cash flows is 10 percent. Does it the IRR change if the Year 1 cash flow is reinvested in the account, or if it is withdrawn and spent on pizza? No. Finally, think back to the yield to maturity calculation on a bond. The YTM is the IRR of the bond investment, but no mention of a reinvestment assumption of the bond coupons is inferred.The reason is that the reinvestment assumption is irrelevant to calculating the YTM on a bond; in the same way, the reinvestment assumption is irrelevant in the IRR calculation.Solutions to Questions and ProblemsNOTE: All end-of-chapter problems were solved using a spreadsheet. Many problems require multiple steps. Due to space and readability constraints, when these intermediate steps are included in this solutions manual, rounding may appear to have occurred. However, the final answer for each problem is found without rounding during any step in the problem.Basic1. a.The payback period is the time that it takes for the cumulative undiscounted cash inflows toequal the initial investment.Project A:Cumulative cash flows Year 1 = €4,000 = €4,000Cumulative cash flows Year 2 = €4,000 +3,500 = €7,500 Payback period = 2 yearsProject B:Cumulative cash flows Year 1 = €2,500 = €2,500Cumulative cash flows Year 2 = €2,500 + 1,200 = €3,700Cumulative cash flows Year 3 = €2,500 + 1,200 + 3,000 = €6,700 Companies can calculate a more precise value using fractional years. To calculate the fractionalpayba ck period, find the fraction of year 3’s cash flows that is needed for the company to have cumulative undiscounted cash flows of €5,000. Divide the difference between the initial investment and the cumulative undiscounted cash flows as of year 2 by the undiscounted cashflow of year 3.Payback period = 2 + (€5,000 –€3,700) / €3,000Payback period = 2.43Since project A has a shorter payback period than project B has, the company should chooseproject A.b.Discount each project’s cash flows at 15 percent. Choose the project with the highest NPV.Project A:NPV = –€7,500 + €4,000 / 1.15 + €3,500 / 1.152 + €1,500 / 1.153NPV = –€388.96Project B:NPV = –€5,000 + €2,500 / 1.15 + €1,200 / 1.152 + €3,000 / 1.153NPV = €53.83The firm should choose Project B since it has a higher NPV than Project A has.2.To calculate the payback period, we need to find the time that the project has recovered its initialinvestment. The cash flows in this problem are an annuity, so the calculation is simpler. If the initial cost is £3,000, the payback period is:Payback = 3 + (£300 / £900) = 3.33 yearsThere is a shortcut to calculate the payback period if the future cash flows are an annuity. Just divide the initial cost by the annual cash flow. For the £3,000 cost, the payback period is:Payback = £3,000 / £900 = 3.33 yearsFor an initial cost of £5,000, the payback period is:Payback = 5 + (£500 / £900) = 5.55 yearsThe payback period for an initial cost of £10,000 is a little trickier. Notice that the total cash inflows after nine years will be:Total cash inflows = 8(£900) = £7,200If the initial cost is £10,000, the project never pays back. Notice that if you use the shortcut forannuity cash flows, you get:Payback = £10,000 / £900 = 11.11 years.This answer does not make sense since the cash flows stop after nine years, so the payback period is never.3.When we use discounted payback, we need to find the value of all cash flows today. The value todayof the project cash flows for the first four years is:Value today of Year 1 cash flow = $7,000/1.14 = $6,140.35Value today of Year 2 cash flow = $7,500/1.142 = $5,771.01Value today of Year 3 cash flow = $8,000/1.143 = $5,399.77Value today of Year 4 cash flow = $8,500/1.144 = $5,032.68To find the discounted payback, we use these values to find the payback period. The discounted first year cash flow is $6,140.35, so the discounted payback for an $8,000 initial cost is:Discounted payback = 1 + ($8,000 – 6,140.35)/$5,771.01 = 1.32 yearsFor an initial cost of $13,000, the discounted payback is:Discounted payback = 2 + ($13,000 – 6,140.35 – 5,771.01)/$5,399.77 = 2.20 yearsNotice the calculation of discounted payback. We know the payback period is between two and three years, so we subtract the discounted values of the Year 1 and Year 2 cash flows from the initial cost.This is the numerator, which is the discounted amount we still need to make to recover our initial investment. We divide this amount by the discounted amount we will earn in Year 3 to get the fractional portion of the discounted payback.If the initial cost is $18,000, the discounted payback is:Discounted payback = 3 + ($18,000 – 6,140.35 – 5,771.01 – 5,399.77) / $5,032.68 = 3.14 years4.To calculate the discounted payback, discount all future cash flows back to the present, and use thesediscounted cash flows to calculate the payback period. Doing so, we find:R = 0%: 4 + (£1,100 / £2,100) = 4.52 yearsDiscounted payback = Regular payback = 4.52 yearsR = 5%: £2,100/1.05 + £2,100/1.052 + £2,100/1.053 + £2,100/1.054 + £2,100/1.055 = £9,091.90 £2,100/1.056 = £1,567.05Discounted payback = 5 + (£9,500 – 9,091.90) / £1,567.05 = 5.26 years R = 15%: £2,100/1.15 + £2,100/1.152 + £2,100/1.153 + £2,100/1.154 + £2,100/1.155 + £2,100/1.156 = £7,947.41; The project never pays back.5. a.The average accounting return is the average project earnings after taxes, divided by theaverage book value, or average net investment, of the machine during its life. The book value of the machine is the gross investment minus the accumulated depreciation.Average book value = (Book Value0 + Book Value1 + Book Value2 + Book Value3 +Book Value4 + Book Value5) / (Economic Life)Average book value = ($16,000 + 12,000 + 8,000 + 4,000 + 0) / (5 years)Average book value = $8,000Average Project Earnings = $4,500To find the average accounting return, we divide the average project earnings by the average book value of the machine to calculate the average accounting return. Doing so, we find:Average Accounting Return = Average Project Earnings / Average Book ValueAverage Accounting Return = $4,500 / $8,000Average Accounting Return = 0.5625 or 56.25%6.First, we need to determine the average book value of the project. The book value is the grossinvestment minus accumulated depreciation.Purchase Date Year 1 Year 2 Year 3 Gross Investment €8,000 €8,000 €8,000 €8,000Less: Accumulated Depreciation 0 4,000 6,500 8,000Net Investment €8,000 €4,000 €1,500 €0 Now, we can calculate the average book value as:Average book value = (€8,000 + 4,000 + 1,500 + 0) / (4 years)Average book value = €3,375To calculate the average accounting return, we must remember to use the aftertax average netincome when calculating the average accounting return. So, the average aftertax net income is:Average aftertax net income = (1 – t c) Annual pretax net incomeAverage aftertax net income = (1 – 0.25) €2,000Average aftertax net income = €1,500The average accounting return is the average after-tax net income divided by the average book value, which is:Average accounting return = €1,500 / €3,375Average accounting return = 0.4444 or 44.44%7.The IRR is the interest rate that makes the NPV of the project equal to zero. So, the equation that definesthe IRR for this project is:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)30 = –¥8,000,000 + ¥4,000,000/(1 + IRR) + ¥3,000,000/(1 + IRR)2 + ¥2,000,000/(1 + IRR)3Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that: IRR = 6.93%Since the IRR is less than the required return we would reject the project.8.The IRR is the interest rate that makes the NPV of the project equal to zero. So, the equation that definesthe IRR for this Project A is:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)30 = – £2,000 + £1,000/(1 + IRR) + £1,500/(1 + IRR)2 + £2,000/(1 + IRR)3Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that: IRR = 47.15%And the IRR for Project B is:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)30 = – £1,500 + £500/(1 + IRR) + £1,000/(1 + IRR)2 + £1,500/(1 + IRR)3Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that: IRR = 36.19%9.The profitability index is defined as the PV of the cash inflows divided by the PV of the cashoutflows. The cash flows from this project are an annuity, so the equation for the profitability index is:PI = C(PVIFA R,t) / C0PI = €41,000(PVIFA15%,7) / €160,000PI = 1.066110. a.The profitability index is the present value of the future cash flows divided by the initial cost.So, for Project Alpha, the profitability index is:PI Alpha = [$300 / 1.10 + $700 / 1.102 + $600 / 1.103] / $500 = 2.604And for Project Beta the profitability index is:PI Beta = [$300 / 1.10 + $1,800 / 1.102 + $1,700 / 1.103] / $2,000 = 1.519b.According to the profitability index, you would accept Project Alpha. However, remember theprofitability index rule can lead to incorrect decision when ranking mutually exclusive projects.Intermediate11. a.To have a payback equal to the project’s life, given C is a constant cash flow for N years:C = I/Nb.To have a positive NPV, I < C (PVIFA R%, N). Thus, C > I / (PVIFA R%, N).c.Benefits = C (PVIFA R%, N) = 2 × costs = 2IC = 2I / (PVIFA R%, N)12. a.The IRR is the interest rate that makes the NPV of the project equal to zero. So, the equationthat defines the IRR for this project is:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)3 + C4 / (1 + IRR)40 = ₩5,000 –₩2,500 / (1 + IRR) –₩2,000 / (1 + IRR)2–₩1,000 / (1 + IRR)3–₩1,000 / (1 +IRR)4Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that:IRR = 13.99%b.This problem differs from previous ones because the initial cash flow is positive and all futurecash flows are negative. In other words, this is a financing-type project, while previous projects were investing-type projects. For financing situations, accept the project when the IRR is less than the discount rate. Reject the project when the IRR is greater than the discount rate.IRR = 13.99%Discount Rate = 12%IRR > Discount RateReject the offer when the discount rate is less than the IRR.ing the same reason as part b., we would accept the project if the discount rate is 20 percent.IRR = 13.99%Discount Rate = 19%IRR < Discount RateAccept the offer when the discount rate is greater than the IRR.d.The NPV is the sum of the present value of all cash flows, so the NPV of the project if thediscount rate is 10 percent will be:NPV = ₩5,000 –₩2,500 / 1.12 –₩2,000 / 1.122–₩1,000 / 1.123–₩1,000 / 1.124NPV = –₩173.83When the discount rate is 12 percent, the NPV of the offer is –₩359.95. Reject the offer.And the NPV of the project is the discount rate is 19 percent will be:NPV = ₩5,000 –₩2,500 / 1.19 –₩2,000 / 1.192–₩1,000 / 1.193–₩1,000 / 1.194NPV = ₩394.75When the discount rate is 19 percent, the NPV of the offer is ₩466.82. Accept the offer.e.Yes, the decisions under the NPV rule are consistent with the choices made under the IRR rulesince the signs of the cash flows change only once.13. a.The IRR is the interest rate that makes the NPV of the project equal to zero. So, the IRR foreach project is:Deepwater Fishing IRR:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)30 = –$600,000 + $270,000 / (1 + IRR) + $350,000 / (1 + IRR)2 + $300,000 / (1 + IRR)3Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that:IRR = 24.30%Submarine Ride IRR:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)30 = –$1,800,000 + $1,000,000 / (1 + IRR) + $700,000 / (1 + IRR)2 + $900,000 / (1 + IRR)3Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that:IRR = 21.46%Based on the IRR rule, the deepwater fishing project should be chosen because it has the higher IRR.b.To calculate the incremental IRR, we s ubtract the smaller project’s cash flows from the largerproject’s cash flows. In this case, we subtract the deepwater fishing cash flows from the submarine ride cash flows. The incremental IRR is the IRR of these incremental cash flows. So, the incremental cash flows of the submarine ride are:Year 0Year 1Year 2 Year 3 Submarine Ride –$1,800,000 $1,000,000 $700,000 $900,000Deepwater Fishing –600,000 270,000 350,000 300,000Submarine – Fishing –$1,200,000 $730,000 $350,000 $600,000 Setting the present value of these incremental cash flows equal to zero, we find the incremental IRR is:0 = C0 + C1 / (1 + IRR) + C2 / (1 + IRR)2 + C3 / (1 + IRR)30 = –$1,200,000 + $730,000 / (1 + IRR) + $350,000 / (1 + IRR)2 + $600,000 / (1 + IRR)3Using a spreadsheet, financial calculator, or trial and error to find the root of the equation, we find that:Incremental IRR = 19.92%For investing-type projects, accept the larger project when the incremental IRR is greater than the discount rate. Since the incremental IRR, 19.92%, is greater than the required rate of return of 15 percent, choose the submarine ride project. Note that this is the choice when evaluating only the IRR of each project. The IRR decision rule is flawed because there is a scale problem.That is, the submarine ride has a greater initial investment than does the deepwater fishing project. This problem is corrected by calculating the IRR of the incremental cash flows, or by evaluating the NPV of each project.c.The NPV is the sum of the present value of the cash flows from the project, so the NPV of eachproject will be:Deepwater fishing:NPV = –$600,000 + $270,000 / 1.15 + $350,000 / 1.152 + $300,000 / 1.153NPV = $96,687.76Submarine ride:NPV = –$1,800,000 + $1,000,000 / 1.15 + $700,000 / 1.152 + $900,000 / 1.153NPV = $190,630.39Since the NPV of the submarine ride project is greater than the NPV of the deepwater fishingproject, choose the submarine ride project. The incremental IRR rule is always consistent withthe NPV rule.14. a.The profitability index is the PV of the future cash flows divided by the initial investment. Thecash flows for both projects are an annuity, so:PI I = 元15,000(PVIFA10%,3 ) / 元30,000 = 1.243PI II = 元2,800(PVIFA10%,3) / 元5,000 = 1.393The profitability index decision rule implies that we accept project II, since PI II is greater thanthe PI I.b.The NPV of each project is:NPV I = –元30,000 + 元15,000(PVIFA10%,3) = 元7,302.78NPV II = –元5,000 + 元2,800(PVIFA10%,3) = 元1,963.19The NPV decision rule implies accepting Project I, since the NPV I is greater than the NPV II.ing the profitability index to compare mutually exclusive projects can be ambiguous whenthe magnitudes of the cash flows for the two projects are of different scale. In this problem,project I is roughly 3 times as large as project II and produces a larger NPV, yet the profit-ability index criterion implies that project II is more acceptable.15. a.The equation for the NPV of the project is:NPV = –₦28,000,000 + ₦53,000,000/1.11 –₦8,000,000/1.112 = ₦13,254,768.28The NPV is greater than 0, so we would accept the project.b.The equation for the IRR of the project is:0 = –₦28,000,000 + ₦53,000,000/(1+IRR) –₦8,000,000/(1+IRR)2From Descartes rule of signs, we know there are two IRRs since the cash flows change signstwice. From trial and error, the two IRRs are:IRR = 72.75%, –83.46%。

公司金融课后习题罗斯

第一章Corporate finance（公司财务）是金融学的分支学科，用于考察公司如何有效地利用各种融资渠道，获得最低成本的资金来源，并形成合适的资本结构（capital structure）；还包括企业投资、利润分配、运营资金管理及财务分析等方面。

它会涉及到现代公司制度中的一些诸如委托-代理结构的金融安排等深层次的问题。

为什么说公司理财研究的就是如下三个问题：(1) 公司应该投资于什么样的长期资产？涉及资产负债表的左边。

我们使用“资本预算(capital budgeting)”和“资本性支出”这些专业术语描述这些长期固定资产的投资和管理过程。

(2) 公司如何筹集资本性支出所需的资金呢？涉及资产负债表的右边。

回答这一问题又涉及到资本结构(Capital structure)，它表示公司短期及长期负债与股东权益的比例。

(3) 公司应该如何管理它在经营中的现金流量？涉及资产负债表的上方。

首先，经营中的现金流入量和现金流出量在时间上不对等。

此外，经营中现金流量的数额和时间都具有不确定性，难于确切掌握。

财务经理必须致力于管理现金流量的缺口。

从资产负债表的角度看，现金流量的短期管理与净营运资本(net working capital)有关。

净营运资本定义为短期资本与短期负债之差。

从财务管理的角度看，短期现金流量问题是由于现金流量和现金流量之间不对等所引起的，属于短期理财问题。

资本结构公司可以事先发行比股权多的债权，筹集所需的资金；可以考虑改变二者的比例，买回它的一些债权。

融资决策在原先投资决策前就可以独立设定。

这些发行债权和股权的决策影响到公司的资本结构。

资金主管负责处理现金流量、投资预算和制定财务计划。

财务主管负责会计工作职能，包括税收、成本核算、财务会计和信息系统。

现金流量的时点公司投资的价值取决于现金流量的时点。

一个最重要的假设是任何人都偏好早一点收到现金流量。

今天收到的一美元比明天收到的一美元更有价值。

公司理财(罗斯)第5章(英文)

2005 The McGraw-Hill Companies, Inc. All Rights Reserved.
McGraw-Hill/Irwin Corporate Finance, 7/e
5-2
Valuation of Bonds and Stock
First Principles:
Value of financial securities = PV of expected future cash flows
5-10
Example of pure discount bonds:
What is the price of a 25-year, pure discount bond that pays $50 at maturity if the current yield-to maturity is 8 percent? 0 1 2… 25 |----------|---------|-------------------| $50 PV0 = 50 ÷ (1.08)25 = $7.30
Time to maturity (T) = Maturity date - today’s date Face value (F) Discount rate (r)
$0
0
$0
$0
$F
T
1
2
T 1
Present value of a pure discount bond at time 0:
F PV = T (1+ r)
批注本地保存成功开通会员云端永久保存去开通
5-0
CHAPTER
5
2005 The McGraw-Hill Companies, Inc. All Rights Reserved.

公司理财-罗斯课后习题答案.pdf

非经济现象,最好的处理方式是通过政治手段。一个经典的思考问题给出了这种争论的答
案:公司估计提高某种产品安全性的成本是 30 美元万。然而,该公司认为提高产品的安全
性只会节省 20 美元万。请问公司应该怎么做呢?”
5.财务管理的目标都是相同的,但实现目标的最好方式可能是不同的,因为不同的国家有不
同的社会、政治环境和经济制度。
有效使用资金以最大限度地实现组织的社会使命。
3.这句话是不正确的。管理者实施财务管理的目标就是最大化现有股票的每股价值，当前
的股票价值反映了短期和长期的风险、时间以及未来现金流量。
4.有两种结论。一种极端,在市场经济中所有的东西都被定价。因此所有目标都有一个最优
水平，包括避免不道德或非法的行为，股票价值最大化。另一种极端,我们可以认为这是
股东的最大化权益行事。现在的管理层经常在公司面临这些恶意收购的情况时迷失自己
的方向。
7.其他国家的代理问题并不严重,主要取决于其他国家的私人投资者占比重较小。较少的私
人投资者能减少不同的企业目标。高比重的机构所有权导致高学历的股东和管理层讨论
决策风险项目。此外,机构投资者比私人投资者可以根据自己的资源和经验更好地对管理
明公司财务有什么异样,但两种方法都没有说明差异是好的还是坏的。例如,假设公司的
流动比率增大，这可能意味着公司改善了过去流动性存在的问题,也可能意味着公司对资
产的管理效率下降。同类公司分析也可能出现问题。公司的流动比率低于同类公司可能
表明其资产管理更有效率,也可能公司面对流动性问题。两种分析方法都没有说明比率的
8.例如,如果一个公司的库存管理变得更有效率,一定数量的存货需要将会下降。如果该公司
可以提高应收帐款回收率，同样可以降低存货需求。一般来说,任何导致期末的 NWC 相