Maths IGCSE Quick Revision

合集下载

igcse数学

igcse数学IGCSE数学是国际通用的GCSE（General Certificate of Secondary Education）课程中的一门科目，其全称是“International General Certificate of Secondary Education Mathematics”，也称作IGCSE数学，其学习内容涵盖了初中和高中数学的最重要的部分，强调对数学知识的深刻理解和应用能力的培养，考察学生的基础知识、数学思维的能力以及解决实际问题的能力。

IGCSE数学在国际上广泛受到认可，且在很多领域都是必要的入门课程。

IGCSE数学的课程包括三个不同的层次：基础、扩展和更高层次。

每个层次由一套统一的考试组成，考生可以选择其中一个层次进行学习和考试。

基础层次旨在培养学生基本的数学知识和技能，适合那些对数学知识和学习不太熟悉的学生；扩展层次则更加强调对数学知识的掌握和应用能力的培养，适合那些想要在高中学习数学的学生；更高层次则是高阶数学学过的内容，适合有强烈数学兴趣和准备以后继续在数学领域发展的学生。

IGCSE数学课程的主要内容包括数学基础知识，如整数、分数、小数、代数、几何、概率和统计学等；数学思维，包括如何分析和解决数学问题以及如何理解和应用数学知识；实际应用能力，即如何将数学知识应用到实际生活和工作中；探究性学习，即鼓励学生进行自主研究和探究，发现和解决复杂的数学问题。

IGCSE数学考试的组成包括两个阶段：核心层次和扩展层次。

核心层次考试涵盖数学基础知识和数学思维能力，共包含两个考试，分别为数学试卷1和数学试卷2，每个试卷各持续1个半小时。

扩展层次考试更加注重数学应用能力和探究性学习，分为数学试卷3和数学试卷4，每个试卷都持续1个半小时。

总的来说，IGCSE数学是一门非常重要的数学课程，可以为学生提供很好的数学思维和实际应用能力的培养，并为学生的未来学习和工作打下坚实的数学基础。

igcse评分等级评分标准

IGCSE评分等级和评分标准是国际通用的标准化考试评价体系，旨在为全球的中学生提供一个公平和可比较的学术评估。

IGCSE评分等级分为A*～G共7个级别，其中A*为最高级别，G为最低级别。

评分标准则根据不同科目和考试要求的不同而有所不同，主要考察学生对知识、概念和应用能力的理解和掌握程度。

我们来详细了解一下IGCSE评分等级的具体标准。

在IGCSE考试中，A*级别是对学生能够充分理解和应用所学知识的最高要求。

学生需要在考试中展现出对知识的深刻理解和高水平的运用能力，能够独立分析和解决复杂的问题。

而G级别则代表学生的知识和能力水平较为薄弱，可能只能简单描述基本概念或运用简单的知识进行简单的分析。

其他等级则介于A*和G之间，对学生的能力和水平有不同程度的要求和评价。

IGCSE评分标准在不同科目中也有所不同。

以数学科目为例，A*级别的学生需要能够熟练运用复杂的数学方法和技巧，能够独立解决较为复杂的数学问题，同时能够展现出对数学概念的深刻理解和应用能力。

而G级别的学生可能只能简单地进行数学计算，对数学概念的理解较为薄弱。

其他科目的评分标准也是根据学科特点和要求进行具体规定的，旨在全面评价学生在不同学科领域的学术能力和水平。

在撰写文章时，我建议你从整体认识IGCSE评分等级和评分标准开始，向读者介绍什么是IGCSE评分等级，每个等级代表什么样的学术能力和水平，以及评分标准的具体内容和要求。

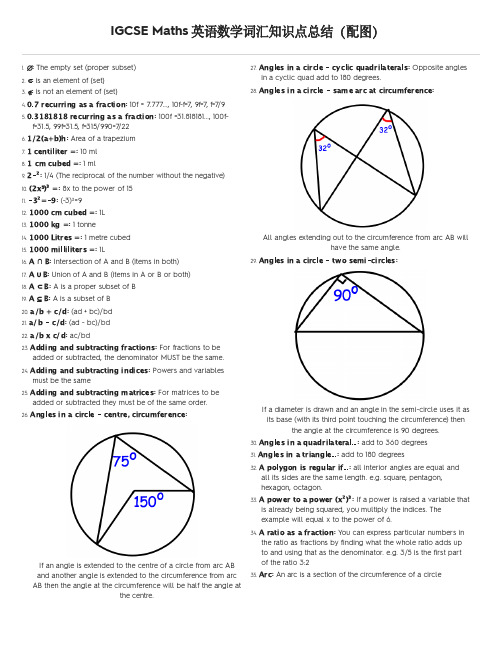
你可以根据具体的学科领域和要求，针对性地展开讨论，介绍不同科目的评分标准和要求是如何设计和规定的，同时可以举例说明，让读者更加直观地理解和了解IGCSE评分等级和评分标准的具体内容。

你可以结合个人观点和理解，对IGCSE评分等级和评分标准进行总结和回顾，展示你对这一主题的深刻认识和理解。

IGCSE评分等级和评分标准是全球通用的标准化考试评价体系，是对学生学术能力和水平的全面评估和认证。

通过详细了解和深入探讨IGCSE评分等级和评分标准的内容和要求，我们能够更加全面、深刻和灵活地理解这一主题，为自己的学习和成长指明方向和目标。

IGCSE Maths ast aper数学考试题

The total mark for this paper is 100. The marks for parts of questions are shown in round brackets:
14
e.g. (2).
You may use a calculator.
15
Advice to Candidates
(b) Use Pythagoras’ Theorem to work out the length of AB. Give your answer correct to 3 significant figures.
(............ , ............) (2)
N20710RA
......................... cm (4) Q6
2 cm 3 cm 6 cm
7 cm
....................... cm2 (2)
Diagram NOT accurately drawn
11 cm The diagram shows a shape. (b) Work out the area of the shape.
N20710RA
Centre No.
Candidate No.
Paper Reference
Surname
4 4 0 0 3 H Signature
Initial(s)
Paper Reference(s)
4400/3H
London Examinations IGCSE
Examiner’s use only Team Leader’s use only
Write your answers neatly and in good English.

gcse数学教材

gcse数学教材
以下是几本常用的GCSE数学教材：
1. Cambridge IGCSE Mathematics Core and Extended Coursebook：剑桥IGCSE数学核心和扩展教材(适用于核心和扩展级别的学生)。

2. Edexcel GCSE Mathematics Student Book：爱德思达GCSE
数学学生书(适用于爱德思达考试板块的学生)。

3. AQA GCSE Mathematics Student Book：AQA GCSE数学学
生书(适用于AQA考试板块的学生)。

4. Oxford Revise GCSE Mathematics Revision Guide：牛津GCSE数学复习指南(适用于复习阶段)。

5. Collins GCSE Mathematics Revision Guide：柯林斯GCSE数
学复习指南(适用于复习阶段)。

请注意，教材选择应根据您所参加的考试板块、学校要求和个人学习风格来进行。

请与您的老师或学校咨询并参考教育机构推荐的教材。

igcse数学课程内容

igcse数学课程内容IGCSE数学课程是一门广泛涵盖数学基础知识和技能的课程，为学生提供了一种全面了解数学概念和应用的机会。

以下是一些与IGCSE数学课程相关的参考内容。

1. 数的基本概念：IGCSE数学课程首先介绍了数的基本概念，包括自然数、整数、有理数和实数。

学生将学习如何进行数的四则运算，并了解不同类型数的属性，如质数、奇数和偶数等。

2. 代数：代数是IGCSE数学课程的重点内容之一。

学生将学习如何使用字母和符号表示未知数，并掌握代数表达式的展开与因式分解。

此外，学生还会学习一些代数技巧，如求解方程和不等式等。

3. 几何学：IGCSE数学课程还包括几何学的内容。

学生将学习几何图形的性质和关系，如三角形、矩形、圆等。

此外，学生还需要掌握测量长度、面积和体积的技巧。

4. 概率与统计学：在IGCSE数学课程中，学生也会学习概率与统计学的基本概念。

他们将学习概率计算和数据分析的方法，并了解如何解读和使用统计数据。

5. 数学建模：IGCSE数学课程还鼓励学生将数学应用于解决实际问题。

学生将学习如何使用数学建模方法来理解和解决现实生活中的各种情况和挑战。

6. 考试准备：IGCSE数学课程还包括考试准备的内容。

学生将了解考试的格式和要求，并完成各种练习和模拟考试，以帮助他们熟悉考试环境和提高应试能力。

除了上述内容，IGCSE数学课程还注重培养学生的问题解决能力、逻辑思维和分析能力。

学生需要学会将数学的概念和方法应用于实际问题，培养数学思维和创造性解决问题的能力。

总结起来，IGCSE数学课程涵盖了数的基本概念、代数、几何学、概率与统计学、数学建模和考试准备等内容。

通过这门课程，学生将掌握数学的基础知识和技能，培养问题解决能力和创造性思维，为将来更高级别的数学学习奠定坚实的基础。

igcse 的评分标准 -回复

igcse 的评分标准-回复IGCSE评分标准是指考试管理机构根据对考生答题质量的评估所制定的一套评分体系。

这个评分体系由一系列准则和标准组成，旨在确保评卷的公正和准确性。

在本文中，我将逐步解释IGCSE评分标准的主要组成部分。

首先，评分标准的一个重要组成部分是所选科目的知识和技能。

IGCSE考试是为了对考生在各个学科的知识和能力进行评估而设计的。

评分标准会考虑到该科目的课程要求，并给予分数。

例如，在数学科目中，考生需要展示对数学概念和技能的理解和运用。

评分标准将根据考生对数学问题的正确性、解题方法、逻辑推理能力等方面进行评估。

其次，IGCSE评分标准也考虑到考生在答题过程中表现出的思维能力。

这包括分析、综合、评估和判断等方面的能力。

评分标准会根据考生的思维过程和解决问题的方法，评估他们在考试中展示的独立性和创造性。

这一方面的评估可以确保评分的客观性和准确性，并帮助考生发展更高层次的思维能力。

此外，IGCSE评分标准还考虑到考生在答题中所展示的组织和表达能力。

这包括清晰的语言表达、合理的段落结构以及逻辑的文章框架等方面。

评分标准会对考生的写作、口语或其他形式的表达进行评估，以判断他们是否能准确地传达信息和观点。

考生的表达能力对于获得更高的评分至关重要。

此外，IGCSE评分标准还会考虑到考生对所强调的技能的有效运用。

不同科目强调不同的技能，如实验设计、网络研究、数据分析等。

评分标准会根据考生在实践中展示这些技能的能力来评估他们。

考生需要能够有效地应用所学技能来解决真实的问题或研究主题。

这种评估方式确保了考生在现实情境中的技能运用能力。

最后，IGCSE评分标准还会考虑到考生在考试中展示的学业态度和情感因素。

这个方面的考量包括参与度、自信心、自律性等方面的评估。

评分标准会评估考生在考试中的参与程度和积极性。

考生展示的积极态度和对学习的热情，可能会影响他们的最终分数。

综上所述，IGCSE评分标准综合考虑了考生在各个学科中展示的知识、技能、思维能力、组织和表达能力、技能运用以及学业态度和情感因素。

国际数学(IGCSE)与苏教版中考数学课程要求与设置比对

国际数学(IGCSE)与苏教版中考数学课程要求与设置比对中图分类号：G688.2文献标识码：A文章编号：ISSN1001-2982（2020）02-140-01一、IGCSE课程简介IGCSE是International General Certificate of Secondary Education的缩写，意为国际化中学教育证书，是剑桥考评的一部分，其证书在全世界大部分地区都会得到承认，也是申请大学的重要依据之一。

IGCSE始终秉着因人而异，因地制宜地观念发展和培养青少年不同的兴趣、爱好和学科特长。

IGCSE一共有64门学科可供选择，因为它的国际化，故有很大一部分的语言类学科，比如德语、法语等等，其中必修的是数学和英语，数学有三种不同的程度可以选择，核心数学（Core)、拓展数学(Extended)、附加数学(Additional)。

核心数学内容比较简单，根据后续高中阶段数学的能力要求，我校在八、九年级选择的是拓展数学。

无锡市中考是初中学业水平考试的简称，中考不仅衡量学生毕业水平，更是升学的重要途径。

中考科目有语文、数学、英语、物理、化学、道德与法制、历史、体育，共八门。

二、试卷卷面分析IGCSE考试满分200分，分两天考，第一天考试卷一，时长90分钟，满分70分。

通常过三、四天后考试卷二，试卷二时长150分钟，满分130分。

两份试卷成绩合计在一起，最后换算成等第，成绩单上记录等第。

三、课程内容难度比对内容苏教版中考国际数学（IGCSE0580）1代数﹡﹡﹡﹡﹡﹡2几何相似和全等部分﹡﹡﹡﹡3函数变量﹡﹡﹡﹡复合函数﹡﹡﹡二次函数及图像﹡﹡﹡﹡﹡4概率统计﹡﹡﹡﹡﹡﹡三角函数（正余弦定理）﹡﹡﹡﹡﹡﹡﹡﹡﹡6圆、扇形、弧长﹡﹡﹡﹡﹡﹡圆里其他定理﹡﹡﹡﹡7向量﹡﹡﹡8集合﹡﹡9图形变换﹡﹡﹡﹡10导函数、导数﹡﹡11计算器不允许允许四、教材、教辅、教具和教学资源比对中国的教材都是国家统一发放的，无锡的中学数学教材由教育部审定的，杨裕前、董伟林主编的，江苏凤凰科学技术出版社出版的《数学》，初中阶段共分六册，每学期一册。

英国IGCSE剑桥初中剑桥高中考试数学专业词汇中英文对照

IGCSE 数学中英对照词汇代数部分1.基础add，plus 加subtract 减difference 差multiply times 乘product 积divide 除divisible 可被整除的divided evenly 被整除dividend 被除数divisor 因子，除数quotient 商remainder 余数factorial 阶乘power 乘方radical sign, root sign 根号round to 四舍五入to the nearest 四舍五入2.有关集合union 并集proper subset 真子集solution set 解集3.有关代数式、方程和不等式algebraic term 代数项like terms, similar terms 同类项5.基本数学概念arithmetic mean 算术平均值weighted average 加权平均值geometric mean 几何平均数exponent 指数，幂base 乘幂的底数,底边cube 立方数，立方体square root 平方根cube root 立方根common logarithm 常用对数digit 数字constant 常数variable 变量inverse function 反函数complementary function 余函数linear 一次的，线性的factorization 因式分解absolute value 绝对值round off 四舍五入6.有关数论natural number 自然数positive number 正数negative number 负数odd integer 奇整数,odd number 奇数even integer, even number 偶数integer, whole number 整数4.有关分数和小数proper fraction真分数improper fraction假分数mixed number带分数vulgar fraction，common fraction普通分数simple fraction简分数complex fraction繁分数numerator分子denominator分母(least)common denominator(最小)公分母quarter四分之一decimal fraction纯小数infinite decimal无穷小数recurring decimal循环小数tenths unit十分位irrational(number)无理数inverse倒数composite number合数reciprocal倒数common divisor公约数multiple倍数(least)common multiple(最小)公倍数(prime)factor(质)因子common factor公因子prime number质数ordinary scale, decimal scale十进制nonnegative非负的tens十位units个位mode众数median中数common ratio公比positive whole number 正整数negative whole number 负整数consecutive number 连续整数real number, rational number 实数,有理数arentheses 括号=32proportion 比例permutation 排列combination 组合table 表格trigonometric function 三角函数unit 单位,位numerical coefficient 数字系数inequality 不等式triangle inequality 三角不等式range 值域original equation 原方程equivalent equation 同解方程等价方程linear equation 线性方程(e.g.5x+6=22) 7.数列arithmetic progression(sequence)等差数列geometric progression(sequence)等比数列8.其它approximate 近似(anti)clockwise(逆)顺时针方向cardinal 基数ordinal 序数direct proportion 正比distinct 不同的estimation 估计，近似几何部分1.所有的角alternate angle 错角corresponding angle 同位角vertical angle 对顶角central angle 圆心角interior angle 角exterior angle 外角supplementary angles 补角complementary angle 余角adjacent angle 邻角acute angle 锐角obtuse angle 钝角right angle 直角round angle 周角straight angle 平角included angle 夹角2.所有的三角形equilateral triangle 等边三角形scalene triangle 不等边三角形isosceles triangle 等腰三角形right triangle 直角三角形oblique 斜三角形inscribed triangle 接三角形solid 立体的cone 圆锥sphere 球体5.有关立体图形cube 立方体，立方数rectangular solid 长方体regular solid/regular polyhedron 正多面体circular cylinder 圆柱体tangent 切线的transversal 截线intercept 截距6.有关图形上的附属物altitude 高depth 深度side 边长circumference, perimeter 周长radian 弧度surface area 表面积volume 体积arm 直角三角形的股cross section 横截面center of acircle 圆心chord 弦radius 半径angle bisector 角平分线diagonal 对角线diameter 直径edge 棱face of a solid 立体的面hypotenuse 斜边3.有关收敛的平面图形，除三角形外semicircle 半圆concentric circles 同心圆quadrilateral 四边形pentagon 五边形hexagon 六边形heptagon 七边形octagon 八边形nonagon 九边形decagon 十边形polygon 多边形parallelogram 平行四边形equilateral 等边形plane 平面square 正方形，平方rectangle 长方形regular polygon 正多边形rhombus 菱形trapezoid 梯形4.其它平面图形arc 弧line, straight line 直线line segment 线段parallel lines 平行线segment of a circle 弧形其它相关词汇cent 美分penny 一美分硬币included side 夹边leg 三角形的直角边median of a triangle 三角形的中线base 底边，底数(e.g.2 的5 次方，2 就是底数) opposite 直角三角形中的对边midpoint 中点endpoint 端点vertex(复数形式vertices)顶点quart 夸脱gallon 加仑(1gallon=4quart)yard 码meter 米micron 微米inch 英寸7.有关坐标coordinate system 坐标系rectangular coordinate 直角坐标系origin 原点abscissa 横坐标ordinate 纵坐标Number line 数轴quadrant 象限slope 斜率complex plane 复平面8.其它plane geometry 平面几何trigonometry 三角学bisect 平分circumscribe 外切inscribe 切intersect 相交nickel 5 美分硬币dime 一角硬币dozen 打(12 个)score 廿(20 个)Centigrade 摄氏Fahrenheit 华氏foot 英尺minute 分(角度的度量单位，60 分=1 度) square measure 平方单位制cubic meter 立方米pint 品脱(干量或液量的单位) perpendicular 垂直Pythagorean theorem 勾股定理congruent 全等的multilateral 多边的。

igcse数学词汇pdf

igcse数学词汇
以下是IGCSE数学中一些常见的词汇：
1.代数式（Algebraic Expression）：数学表达式，由数字、变量和运算符组成。

2.方程（Equation）：表示两个数学表达式相等。

3.函数（Function）：一个变量依赖于另一个变量的关系。

4.指数（Exponent）：表示一个数被另一个数的次数。

5.系数（Coefficient）：在代数式中，与变量相乘的数。

6.变量（Variable）：可以取不同值的符号。

7.算术运算（Arithmetic Operations）：加、减、乘、除等基本数学运算。

8.三角函数（Trigonometric Functions）：正弦、余弦、正切等函数。

9.平面几何（Plane Geometry）：研究二维形状和图形的学科。

10.立体几何（Solid Geometry）：研究三维形状和图形的学科。

以上是一些IGCSE数学中常见的词汇。

igcse 剑桥评分标准

igcse 剑桥评分标准IGCSE剑桥评分标准：深入了解各项评分准则及应对策略在IGCSE剑桥考试中，评分标准旨在全面评估学生的知识、技能和理解能力。

各个科目都有相应的评分准则，以下我们将详细解析IGCSE英语、数学、科学等热门科目的评分标准，以帮助大家更好地了解考试要求和应对策略。

1. IGCSE英语IGCSE英语考试包括听力、阅读、写作和口语四个部分。

评分时，考官会综合考虑学生的语言准确性、流利程度、词汇量、语法知识、发音、语调等方面。

-听力：听力部分评分主要关注学生对语音、语调、速度和词汇的识别能力，以及能否准确理解题目要求。

-阅读：阅读部分评分关注学生对文章内容的理解程度、推理能力、词汇量和阅读速度。

-写作：写作部分评分重点在于学生的表达能力、逻辑思维、语法运用、词汇运用和文体风格。

-口语：口语部分评分包括语言准确性、流利程度、发音、词汇量、语法知识、话题展开能力和互动能力。

2. IGCSE数学IGCSE数学评分标准涵盖计算能力、问题解决能力、数学概念理解和应用等方面。

评分时，考官会关注学生的解题方法、步骤、数据处理和结论。

-计算能力：评分重点在于学生的计算熟练程度、正确性和速度。

-问题解决：评分关注学生能否运用数学知识解决实际问题，包括理解问题、找到关键信息、运用合适的方法和策略等。

-数学概念理解和应用：评分着重于学生对数学概念的理解程度、能否运用所学知识解决题目以及知识点的灵活运用。

3. IGCSE科学IGCSE科学评分标准包括实验技能、观察能力、数据分析、理论知识掌握和应用等方面。

-实验技能：评分关注学生在实验中的操作熟练程度、安全意识、实验设计和实施能力。

-观察能力：评分依据学生对实验现象的观察准确性、细节把握和描述能力。

-数据分析：评分着重于学生能否正确处理实验数据、分析结果、得出结论。

-理论知识掌握和应用：评分标准包括学生对科学理论的理解程度、能否运用所学知识解决题目以及知识点的灵活运用。

IGCSE math 数学试卷0580_s17_qp_43

This document consists of 19 printed pages and 1 blank page.DC (NH/JG) 130218/2© UCLES 2017[Turn over*0731247115*MATHEMATICS 0580/43Paper 4 (Extended) May/June 20172 hours 30 minutesCandidates answer on the Question Paper.Additional Materials: Electronic calculator Geometrical instrumentsTracing paper (optional).READ THESE INSTRUCTIONS FIRSTWrite your Centre number, candidate number and name on all the work you hand in.Write in dark blue or black pen.You may use an HB pencil for any diagrams or graphs.Do not use staples, paper clips, glue or correction fluid.DO NOT WRITE IN ANY BARCODES.Answer all questions.If working is needed for any question it must be shown below that question.Electronic calculators should be used.If the degree of accuracy is not specified in the question, and if the answer is not exact, give the answer to three significant figures. Give answers in degrees to one decimal place.For π, use either your calculator value or 3.142.At the end of the examination, fasten all your work securely together.The number of marks is given in brackets [ ] at the end of each question or part question.The total of the marks for this paper is 130.Cambridge International ExaminationsCambridge International General Certificate of Secondary Education1 (a) In 2016, a company sold 9600 cars, correct to the nearest hundred.(i) Write down the lower bound for the number of cars sold. (1)(ii) The average profit on each car sold was $2430, correct to the nearest $10.Calculate the lower bound for the total profit.Write down the exact answer.$ (2)(iii) Write your answer to part (a)(ii) correct to 4 significant figures.$ (1)(iv) Write your answer to part (a)(iii) in standard form.$ (1)(b) In April, the number of cars sold was 546.This was an increase of 5% on the number of cars sold in March.Calculate the number of cars sold in March. (3)© UCLES 20170580/43/M/J/17(c) The price of a new car grows exponentially by 3% per year.A new car has a price of $3000 in 2013.Find the price of a new car 4 years later.$ (2)© UCLES 2017[Turn over0580/43/M/J/170580/43/M/J/17© UCLES 20172 (a)y °x °z °DABPQSRCE NOT TO SCALE24°38°PQ is parallel to RS .ABC and ADE are straight lines. Find the values of x , y and z .x = ..................................................y = ..................................................z = ..................................................[3] (b)ABDCNOT TOSCALE42°The points A , B , C and D lie on the circumference of the circle. AB = AD , AC = BC and angle ABD = 42°. Find angle CAB .Angle CAB = (3)(c)NOT TOSCALEThe points P, Q, R and S lie on the circumference of the circle, centre O.Angle QOS =146°.Find angle QRS.QRS = . (2)Angle© UCLES 2017[Turn over0580/43/M/J/170580/43/M/J/17© UCLES 20173The table shows some values for 24y x x 32=+.x –2.2–2–1.5–1–0.500.50.8y–1.940.753.58(a) Complete the table.[4](b) Draw the graph of 24y x x 32=+ for 2.20.8x G G - .[4] (c) Find the number of solutions to the equation 243x x 32+=. (1)0580/43/M/J/17© UCLES 2017[Turn over(d) (i) The equation 241x x x 32+-= can be solved by drawing a straight line on the grid.Write down the equation of this straight line.y = ..................................................[1] (ii) Use your graph to solve the equation 241x x x 32+-=.x = ............................ or x = ............................ or x = ............................[3] (e) The tangent to the graph of 24y x x 32=+ has a negative gradient when x k =. Complete the inequality for k ....................... 1 k 1 . (2)0580/43/M/J/17© UCLES 20174 (a) The diagram shows a solid metal prism with cross section ABCDE .BGFKJ DCAEH2 cm7 cm4 cm8 cm 4 cmNOT TOSCALE(i) Calculate the area of the cross section ABCDE .............................................cm 2 [6](ii) The prism is of length 8 cm.Calculate the volume of the prism.............................................cm 3 [1](b) A cylinder of length 13 cm has volume 280 cm3.(i) Calculate the radius of the cylinder..............................................cm [3] (ii) The cylinder is placed in a box that is a cube of side 14 cm.Calculate the percentage of the volume of the box that is occupied by the cylinder................................................% [3]© UCLES 2017[Turn over0580/43/M/J/175 (a) Haroon has 200 letters to post.The histogram shows information about the masses, m grams, of the letters.Mass (grams)mFrequencydensity(i) Complete the frequency table for the 200 letters.Mass (m grams)0 1m G 1010 1m G 2020 1m G 2525 1m G 3030 1m G 50Frequency5017[3](ii) Calculate an estimate of the mean mass.................................................g [4]0580/43/M/J/17© UCLES 2017(b) Haroon has 15 parcels to post.The table shows information about the sizes of these parcels.Size Small LargeFrequency96Two parcels are selected at random.Find the probability that(i) both parcels are large, (2)(ii) one parcel is small and the other is large. (3)(c) The probability that a parcel arrives late is 803.4000 parcels are posted.Calculate an estimate of the number of parcels expected to arrive late. (1)6(a) Describe fully the single transformation that maps shape A onto(i) shape B,...................................................................................................................................................... (2)(ii) shape C....................................................................................................................................................... (3)(b) Draw the image of shape A after rotation through 90° anticlockwise about the point (3, -1). [2]y=. [2] (c) Draw the image of shape A after reflection in 1f p.(d) Describe fully the single transformation represented by the matrix 3003.............................................................................................................................................................. (3)7 (a) Solve the simultaneous equations. You must show all your working.x y 2311+=x y 3550-=- x = ..................................................y = (4)(b) 12x x a x b 22-+=+^h Find the value of a and the value of b .a = ..................................................b = (3)(c) Write as a single fraction in its simplest form.x x x x 25132-+-+ (4)8 (a)The table shows the marks gained by 10 students in their physics test and their mathematics test.The first six points have been plotted for you.MathematicsmarkPhysics mark[2](ii) What type of correlation is shown in the scatter diagram? (1)(b) The marks of 30 students in a spelling test are shown in the table below.Mark012345Frequency245568Find the mean, median, mode and range of these marks.Mean = ..................................................Median = ..................................................Mode = ..................................................Range = (7)(c) The table shows the marks gained by some students in their English test.Mark 527591Number of students x4511The mean mark for these students is 70.3 .Find the value of x.= (3)x9ACQ B525 m872 m104°NOT TO SCALEABC is a triangular field on horizontal ground. There is a vertical pole BQ at B .AB = 525 m, BC = 872 m and angle ABC = 104°.(a) Use the cosine rule to calculate the distance AC .AC = ..............................................m [4] (b) The angle of elevation of Q from C is 1.0°.Showing all your working, calculate the angle of elevation of Q from A . (4)(c) (i) Calculate the area of the field.............................................. m2 [2] (ii) The field is drawn on a map with the scale 1 : 20 000.Calculate the area of the field on the map in cm2.............................................cm2 [2]10 = {21, 22, 23, 24, 25, 26, 27, 28, 29, 30} A = { x : x is a multiple of 3} B = { x : x is prime} C = { x : x G 25}(a) Complete the Venn diagram.ABCᏱ[4] (b) Use set notation to complete the statements. (i) 26 ..................... B [1](ii) A + B = .....................[1](c) List the elements of B , (C + A ). (2)(d) Find (i) n(C ),...................................................[1] (ii) B B C n ,+l ^^h h ....................................................[1] (e)A C +^h is a subset of A C ,^h . Complete this statement using set notation.A C +^h ..................... A C ,^h [1]11 The table shows the first four terms in sequences A, B, C and D.Complete the table.BLANK PAGEPermission to reproduce items where third-party owned material protected by copyright is included has been sought and cleared where possible. Every reasonable effort has been made by the publisher (UCLES) to trace copyright holders, but if any items requiring clearance have unwittingly been included, the publisher will be pleased to make amends at the earliest possible opportunity.To avoid the issue of disclosure of answer-related information to candidates, all copyright acknowledgements are reproduced online in the Cambridge International Examinations Copyright Acknowledgements Booklet. This is produced for each series of examinations and is freely available to download at after the live examination series.Cambridge International Examinations is part of the Cambridge Assessment Group. Cambridge Assessment is the brand name of University of Cambridge Local Examinations Syndicate (UCLES), which is itself a department of the University of Cambridge.。

IGCSE数学词汇chapter 1

IGCSE-MathematicsChapter 1: Reviewing number concepts一：Glossary&Expressions1.1Different types of numbers1.natural number [ 数] 自然数（5 星）2.whole number 整数（5 星）3.even number [ 数] 偶数（5 星）4.odd number [ 数] 奇数（5 星）5.integer ['intid ʒə] n. [ 数] 整数；整体；完整的事物（5 星）6.prime number [ 数] 素数（5 星）7.prime [praim] adj. 主要的；最好的；基本的（4 星）8.square [skwεə] n. 平方；正方形adj. 平方的；正方形的；直角的；正直的vt. 使成方形； vi. 成方形 adv. 成直角地（5 星）9.square number [ 数] 平方数（5 星）10.fraction ['frækʃən] n. 分数；部分；小部分；（5星）11.negative['neɡət iv]n.负数；adj.[数]负的；（5星）12.negative integers 负整数（5 星）13.consecutive [k ən'sekjutiv] adj. 连贯的；连续不断的（5 星）mon fraction [ 数] 普通分数； [数] 简分数（5 星）15.decimal ['desim əl] n. 小数adj. 小数的；十进位的（5 星）16.is equal to 等于（5 星）17.equal ['i:kw əl] n.相等的事物adj. 平等的；相等的；vt. 等于；比得上（5 星）18.is not equal to 不等于（5 星）19.is approximately e qual to 约等于（4 星）20.approximately [ ə'prɔksimitli] adv. 大约，近似地；近于（4 星）21.is less than 小于（5 星）22.is less than or e qual to 小于等于（5 星）23.is greater than 大于（5 星）24.is greater than or equal to 大于等于（ 5 星）25.therefore ['ðεəfɔ:] adv. 因此；所以（4 星）26.square root of 的算术平方根（5 星）27.add [æd] n. 加法，加法运算vi. 加；增加；加起来；做加法vt. 增加，添加；计算…总和（5 星）28.addition [ ə'diʃən] n. 添加；[数] 加法；增加物（5 星）29.mi nus ['main əs] prep. 减，减去 n. 负号，减号；不足；负数 adj. 减的；负的（5星）30.subtract [s əb'trækt] vt. 减去；（5 星）31.subtraction [s əb'trækʃən] n. [ 数] 减法；减少；差集（5 星）32.t im e s[t aim z]n.时代（time的复数）；[数]次数p re p.乘以v.使…相乘（5星）33.multiply ['m ʌltiplai] adj. 多层的；多样的 vt. 乘；使增加；使相乘 vi. 乘；增加（5 星）34.divide[di'vaid]vt.除；（5星）35.divided by 除以（5 星）36.sum [s ʌm] n. 总数 vt. 总结；合计 vi. 概括（5 星）37.difference ['dif ərən s] n. 差异；不同；（5 星）38.product ['pr ɔdək t, - ʌkt] n. 产品；结果； [数] 乘积；（5星）39.quotient ['kw əuʃənt] n. [ 数] 商；系数；（5 星）40.remai n d e r [ri'meind ə] n. [ 数] 余数，残余；剩余物； adj. 剩余的；吃剩的 vt. 廉价出售；削价出售vi. 廉价出售；削价出售（5 星）41.divisible [di'viz əbl] adj. 可分的；可分割的（5 星）42.reciprocal[ri'sipr əkəl] n. [ 数] 倒数； adj. 倒数的（5 星）43.finding 找，求1.2Multiples and factors1.multiples ['m ʌltiplz] n. [ 数] 倍数；（5星）2.factors n. 因素（factor 的复数）v.分解…的因子；（5 星）mon multiple 公倍；[数] 公倍数（5 星）4.the lowest common mulitiples(LCM) 最小公倍数（5 星）mon factors [ 数] 公因数（5 星）6.the highest common factor （HCF ）最大公约数（ 5 星）1.3Prime numbers1.prime numbers 质数；素数（5 星）posite ['k ɔmp əz it] n. 复合材料；合成物；adj. 复合的；合成的；vt. 使合成；使混合（5 星）posite numbers 【数学】合数， (区别于 prime number) （5 星）4.prime factors 质因子（5 星）5.division [di'vi ʒən] n. [ 数] 除法；（5 星）6.divisibility [di,vizi'bil əti] n. 可分性；[数] 可除性（5 星）7.digits ['did ʒits] n. [ 计] 数字；（5 星）1.4P owers and r oots1.powers n.幂（p o we r的复数形式）；（5星）2.roots n. [ 数][植] 根；（4 星）3.square root [ 数] 平方根；二次根（5 星）4.symbol ['simb əl] n. 象征；符号；标志（4 星）5.cube [kju:b] n. 立方；立方体；骰子 vt. 使成立方形；使自乘二次；量…的体积（5星）6.cube numbers 立方数（5 星）7.cube roots [ 数] 立方根（5 星）1.5Working with directed n umbers1.directed numbes 有向数（4 星）2.directed[di'rektid, dai-] v. 指导；管理（d irect 的过去式和过去分词）adj. 定向的；（4 星）3.order [' ɔ:də] n. 命令；顺序；规则；（4 星）4.number line 实数直线；数值轴（5 星）5.ascending [ ə'sendiŋ] adj. 上升的 v. 上升；（5 星）6.descending [di'sendiŋ] n. 递减；下行v. 下降 adj. 下降的；下行的（5 星）1.6Order of operations1.operations n.运营；运作；（5星）2.division [di'vi ʒən] n. [ 数] 除法；（5 星）3.multiplication [,m ʌltipli'kei ʃən] n. [ 数] 乘法；增加（5 星）4.addition [ ə'diʃən] n. 添加；[数] 加法；增加物（5 星）5.subtraction [s əb'trækʃən] n. [ 数] 减法；减少；差集（5 星）6.brac k e t s[b rækət s]n.方括号；（5星）7.power [pau ə] n. [ 数] 幂（3 星）8.working with 使用（3 星）9.working with brackets 使用括号（ 3 星）10.BODMAS （bracket, of, divide, multiply, add, subtract ）(5 星)1.7Rounding numbers1.rounding n. [ 数]凑整；v. 把…弄成整数[数]凑整的；环绕的（4 星）2.rounding numbers 四舍五入（4 星）3.accuracy ['ækjur əs i] n. [ 数] 精确度，准确性（4 星）4.correct to 取值至；准确至；精确到（5 星）5.decimal place [ 数] 小数位（5 星）6.significant [sig'nifik ənt] adj. 重大的；有效的；有意义的；（4 星）7.significant figures [ 计] 有效数字（5 星）8.round down 下舍入（4 星）9.round up 上舍入（4 星）10.standard form 标准形式科学记数法（5星）11.calculator['kælkjulei tə]n.计算器；（5星）12．calculator use 用计算器（3 星）。

igcse数学知识点总结

igcse数学知识点总结igcse学是世界上最常考的数学考试之一，也是很多学生在准备考试过程中最重要的一部分。

准备igcse数学的过程中，学生们必须掌握其中的大量知识点，深入理解和操练，以保证能达到优异的考试成绩。

本文将集中讨论igcse数学知识点，为学生们提供参考。

igcse数学首先涉及到几何方面。

几何包含很多概念，例如：几何图形的周长和面积；三角形的内角和外角的和；四边形的属性，如角平分线、对角线、角的长度等；几何图形的轴对称性和镜像对称性；直角三角形的勾股定理；坐标系等等，学生需要熟练掌握这些知识点。

igcse数学还包括基本的代数，主要包括方程和不等式等，学生需要清楚地理解这些知识点，要会在复杂的实际情况中运用其中的概念来求解方程，并能正确推导出结果。

此外，igcse数学还包括数据分析和概率统计的知识，例如：使用表格、图形和统计指标来组织和分析数据；概率定义；概率计算；伯努利概率；独立性、互斥性；伯努利实验；均值、方差；概率分布和随机变量等，学生要深入理解这些概念和计算方法，以便在考试中熟练运用。

最后，igcse数学还涉及到微积分等方面的知识，学生们需要掌握常用的微积分概念，包括：微分、积分、微分方程等，并要深入理解与之相关的一些经典定理及其证明，同时要熟练掌握与之相关的计算方法，以备考试之用。

总之，igcse数学考试涉及到很多方面的知识点，如几何、代数、数据分析和概率、微积分等等，要想取得理想的成绩，学生们必须掌握这些知识点，努力学习，不断复习，多练习，努力达到理想的考试成绩。

igcse数学考试的准备对学生来说是一项重要的任务。

上述就是igcse数学知识点的总结，希望能给学生们提供帮助。

虽然数学考试是一门困难的学科，但只要认真学习，勤加练习，克服困难，一定能取得理想的成绩。

IGCSE_Revision_Guide_Answers_physics

b) i) weight and tension ii) Towards centre of circle c) PE = mgh = 0.2100.05 = 0.1J 3 a)
b) a = ∆v = 12 = 1.5 m/s² t 8 c) distance = ½512 = 30m d) f=mxa = 4000 1.2 = 4800N e) For the same accelerating force, there is a greater mass due to the extra passengers and so acceleration is less. 4 a) i)
A Page 53 1
0.0003
a) Constant random motion b)As the temperature increases, the velocity of the particles increases. Particles collide with walls of container more often and with greater force and so the pressure increases. a) Evaporation b) Particles with greatest ICE escape from surface of water, reducing the volume of water.
d = 2 × radius = 2r Area = r² Volume = r²l Density = r²l m 3 a) Volume = Reading Q – Reading P b) Mass = Reading S – Reading R c) i) P = m v ii) p = 57.5 = 2.3 g/cm³ 25 Page 17 1 l = 3.6 cm 2 A = r² - (d)² = (0.6)² = 0.28 cm² 2 2 3 V = r²l = 1.0 cm³ Volume of bundle – 10 × 10 – 10.0 cm³ P = m = 59.1 – 5.91 g/cm³ v 10.0 Page 21 1 a) length = 17.5 cm b) load = 2.25 N

IGCSE Maths 英语数学词汇知识点总结(配图)

1.∅: The empty set (proper subset)2.∈: is an element of {set}3.∉: is not an element of {set}4.0.7 recurring as a fraction: 10f = 7.777..., 10f-f=7, 9f=7, f=7/95.0.3181818 recurring as a fraction: 100f =31.818181..., 100f-f=31.5, 99f=31.5, f=315/990=7/226.1/2(a+b)h: Area of a trapezium7.1 centiliter =: 10 ml8.1 cm cubed =: 1 ml9.2-²: 1/4 (The reciprocal of the number without the negative)10.(2x⁵)³ =: 8x to the power of 1511.-3²=-9: (-3)²=912.1000 cm cubed =: 1L13.1000 kg =: 1 tonne14.1000 Litres =: 1 metre cubed15.1000 milliliters =: 1L16.A ∩ B: Intersection of A and B (items in both)17.A ∪ B: Union of A and B (items in A or B or both)18.A ⊂ B: A is a proper subset of B19.A ⊆ B: A is a subset of B20.a/b + c/d: (ad + bc)/bd21.a/b - c/d: (ad - bc)/bd22.a/b x c/d: ac/bd23.Adding and subtracting fractions: For fractions to beadded or subtracted, the denominator MUST be the same. 24.Adding and subtracting indices: Powers and variablesmust be the same25.Adding and subtracting matrices: For matrices to beadded or subtracted they must be of the same order.26.Angles in a circle - centre, circumference:If an angle is extended to the centre of a circle from arc ABand another angle is extended to the circumference from arc AB then the angle at the circumference will be half the angle atthe centre.27.Angles in a circle - cyclic quadrilaterals: Opposite anglesin a cyclic quad add to 180 degrees.28.Angles in a circle - same arc at circumference:All angles extending out to the circumference from arc AB willhave the same angle.29.Angles in a circle - two semi-circles:If a diameter is drawn and an angle in the semi-circle uses it as its base (with its third point touching the circumference) then the angle at the circumference is 90 degrees.30.Angles in a quadrilateral...: add to 360 degrees31.Angles in a triangle...: add to 180 degrees32.A polygon is regular if...: all interior angles are equal andall its sides are the same length. e.g. square, pentagon,hexagon, octagon.33.A power to a power (x²)³: If a power is raised a variable thatis already being squared, you multiply the indices. Theexample will equal x to the power of 6.34.A ratio as a fraction: You can express particular numbers inthe ratio as fractions by finding what the whole ratio adds up to and using that as the denominator. e.g. 3/5 is the first part of the ratio 3:235.Arc: An arc is a section of the circumference of a circleIGCSE Maths英语数学词汇知识点总结（配图）36.Area and volume scale factor: Used in similar shapes. Thisapplies the same principles as the linear scale factor but issquared or cubed. To find the linear scale factor from these, find the square or cube root.37.area of cross-section x length: volume of a prism38.a/sinA=b/sinB=c/sinC: The sine rule (can be inverted sosines are on top)39.Average speed: Distance/time40.ax to the power of -5: a/x to the power of 541.Bar charts:All bars are the same width, the heights represent frequency. A dual chart is sometimes useful to compare two bar charts withsimilar data.42.base x height: Area of a square, rectangle and parallelogram43.Calculating probability: Probability can be found with aprobability fraction. The fraction of P(outcome) is the number of ways the outcome can happen/the total number of possible outcomes. This can then be changed to a decimal orpercentage.44.Calculating the angles of a pie chart: To find what angle asector will be, take the frequency divided by the total number and then times that by 360. These angles do not have tolabelled on the chart45.Choosing the right rule - two angles and a side: Sinerule46.Choosing the right rule - two sides and included angle:Cosine rule followed by sine rule47.Chords:A chord is a line in a circle where both ends touch thecircumference, cutting a section of the bined frequency of two events: You can illustrate thepossibilities of two combined events on a possibility diagram where all the possible outcomes for one variable is on oneaxis and for the other on the other axis. Every possibleoutcome for both variables is then represented on the diagram and the probability can be found.posite function expressions: To make an expressionfor the composite function fg(x), put the expression for g, in the place of x in the expression for f. "Take x, apply g, thenapply f"posite functions: When functions are put together, readthem backwards. e.g. fg(2) means start with 2, apply g thenapply fpound interest: amount x 1. percentage to number ofyearspound interest formula: P(1+r/100)^n where r is thepercentage, n is the time and P is the amount invested.53.Congruent shapes: Shapes with the same size and shape aseach other are congruent, even if they are placed in different positions.54.Construct a perpendicular from a point to a line: Withthe point as the centre, draw an arc that intersects the line on either side. Using these points as the centre, follow the same directions as constructing a perpendicular.55.Constructing a line bisector:Open compass to abut three quarters the length of the line and draw a semi-circle arc from either point on the line. The bisector is the point where the two arcs intersect.56.Constructing an accurate trapezium: To construct atrapezium accurately, use first a protractor and a ruler andthen a set square to find the parallel side.57.Constructing an angle bisector:With the vertex of the angle as the centre, draw an arc through both lines and then, with centres where the arc intersects both lines, draw two more arcs. The angle bisector extends from the vertex to the point where the two arcs intersect.58.Constructing a triangle: Draw the longest side as the base.Draw the next longest side with a compass as an arc and do the same with the shortest side. The draw the straight line to where the two arcs intersect.59.Continuous data: Data that can have any value within a rangeof values, for example mass, height, time.60.CosA= b squared+c squared - a squared/2bc:The cosine rule61.Cosine rule notes: b and c in the rule are the sides makingthe angle being calculated and a is the opposite side. Is not restricted to right-angled triangles62.Cubic sequences: Compare to cube number first. Differencebetween the second difference is the same (3rd difference). 1/6 of the 3rd difference will be the coefficient of n cubed. Take that away from the original sequence and find the rule for the remaining numbers.63.Cumulative frequency diagrams: A frequency table wherethe next the value has been added on to the previous value so that the number is always rising. This can then be plotted with the top value of the class group against the cumulativefrequency. Plots can be joined with a freehand curve.64.Currency conversions: Use value that is not one for bothexchanges (either times by for currency represented by 1 ordivide by for currency represented by point something)65.Decreases in ratios: Decrease 450 in the ratio 5:3. 450=5parts, need to find 3 parts. Decreased amount = (3/5) x 450 66.Describing translations with vectors: As shapes that havebeen translated are congruent, all points have moved thesame amount. The translation is how any one point movedfrom its original place to its new place with its movementacross on top and up and down on the bottom of the fraction without a line.67.Diﬀerent form of function notation - f:x→3x-5: This isthe same as f(x)=3x-568.Discrete data: This is data which contains separate numberslike goals scored, number of children or shoe size.69.Dividing a quantity in a ratio: To divide a quantity in a ratio,you must first find the total number of parts. Divide thequantity by the total number of parts to find one part and then multiply this to find parts of the ratio.70.Dividing fraction: first term x reciprocal of second term71.Dividing fractions: To divide fractions, times the first fractionby the reciprocal (turned upside down) of the second.72.Dividing with indices: Subtract indices, numbers stay73.ℰ: Universal set74.Enlarging a shape: An enlargment will occur about a pointand the distance away from this point should be taken intoconsideration as well as the size. The lengths of each side can be easily found by multiplying the original length by the scale factor.75.Enlarging a shape - ray method: If you need to find thepoint from which the shape was enlarged, the ray method ishelpful. Draw lines through corresponding points on the shape.The point where these lines meet is the point from which the shape was enlarged.76.Equilateral triangular prism: It has four planes of symmetry.One from each of the points lengthways and one widthways halfway down. It also has two axis of rotational symmetry. 77.Estimating gradients: You can estimate the gradient of acurved line by drawing a tangent to it and finding the gradient of that line.78.Estimating the median from a cumulative frequencydiagram: The median can be found by looking at the mark on the vertical axis which is half of the total. Follow this along to meet the curve and the value on the horizontal axis down from this is an estimate of the median. The median is also the 50th percentile79.Exponential graphs: This graph will have a changing gradient80.Exponential growth: Use same formula as compoundinterest81.Exponential growth and decay: Use compound interestprinciples82.Exterior angles: The sum of the exterior angles of anypolygon is 360 degrees83.Factorising quadratic equations with coeﬃcients(ax²+bx+c): Write out the factors of a and c. Find the pair ofa factors that multiply with the pair of c factors to make twonumbers that add to b.84.Factorising simple quadratic equations (x²+bx+c):Factorise in the morning - AM stands for add, multiply. Findtwo numbers that add to the second number and multiply to the third number.85.Factorising tips: Factor out ALL common factors even ifthese are brackets, numbers and multiple variables.86.F angles on parallel lines:Called corresponding angles. They are equal87.Finding a matrix transformation: To do this, you only needto find the vector for two points. Find where 1 0 (over eachother) and 0 1 (over each other) will end up and put thevectors for these together in a matrix. Put the image vector of the vector that started on the x-axis first.88.Finding the area of a triangle using sine: 1/2 ab sinC (twosides with included angle)89.Finding the interquartile range.: The interquartile range canbe found by taking the upper quartile (75th percentile, 75%)and taking away the lower quartile (25th percentile, 25%) togive the middle 50%. The upper and lower quartile can befound in the same way as the median but by finding 1/4 and3/4 of the data instead of 1/2.90.Finding the inverse of a 2 x 2 matrix: Of matrix abcd,swap the positions of a and d and change the signs of b and c.Then divide all the numbers by the determinant.91.Finding the multiplier: A multiplier is the percentage dividedby 100 to find the decimal. However to decrease a quantity by 7%, the multiplier used will be 0.93. If a quantity has beendecreases by 7%, the amount left will be 93% of the total 92.Finding the points of a quadratic graph: Draw out a tableand in each row put a separate component of the equation and the answer, then add these all together to find the points. 93.Frequency table:A tally chart of the all the options. The first column is theoptions or numbers you are tallying, the second is the tallys and the third is the numerical value of the number of tallys inthe second column.94.Function notation: f(x)=3x-695.Histograms: Histograms are similar to bar charts except,there are no gaps between bars, the horizontal axis has acontinuous scale, the area of each bar represents thefrequency.96.Histograms with bars of unequal width: Frequency isrepresented by area. THe vertical axis should read frequency density. To find the height of a bar where class widths aredifferent, use the formula: frequency density=frequency of class interval/width of class interval.97.Histograms with unequal width - proportionality: Areasare proportional to frequencies98.If the determinant of a matrix is 0...: It is a singular matrixand the inverse does not exist99.Increase or decrease by a percentage: Find the multiplier(24%=0.24)100.Increases in ratios: Increase 450 in the ratio 5:3. 450 = 3 parts, need to find 5 parts. Increased amount = (5/3) x 450 101.Integers: Whole, positive, negative102.Interior angles: The sum of the interior angles of any polygon can be found using 180 x (n-2) where n is the number of sides103.Inter-quartile range: Bases the measure of spread on the middle 50% of the data removing extreme values104.Inverse functions - f-¹(x): To find the inverse of a function, replace f(x) with y and rearrange the equation to make x the subject. In the final answer, change the y to x.105.Kite:Two pairs of equal adjacent sides. Has one long diagonal and one short diagonal that bisect each other at right angles. The opposite angles between the sides of different lengths areequal.106.length x width x height: volume of a cuboid107.Linear scale factor: Used in similar shapes. To find it, find two corresponding sides and put the bigger side as thenumerator of a fraction. Simplify the fraction.108.Linear sequences: Look for: difference between each number in sequence, difference between that and the first term 109.Line of symmetry (mirror line): A line that can be drawn through a shape so that what can be seen on one side is amirror image of what can be seen on the other side.110.Locus (loci): A locus is a shape or a line which is always the same distance from a given point or line. You may be alsoasked to draw a locus that is equidistant from two points. This will usually be a straight line.111.Map scales: 1:900000 (1cm on map = 900000cm on ground).1cm = 9km. Actual distance km = distance on map cm x 9km 112.Mean: The mean is the sum of all the values in a set divided by the total number of values in the set. IT uses all the values although extreme values can affect it and it has to becalculated.113.Median: The middle value when put in order of size. It is not heavily affected by extreme values and is easy to find forungrouped data. But, it doesn't use all the values and can be hard to understand.114.Mensuration: The part of geometry concerning lengths, areas and volumes115.Mode: The value that occurs most often in a set of data. It can be used for qualitative data, is easy to find and is not affected by extreme values. But, it doesn't use all the values and may not exist.116.Multiplying fractional indices: Fractional indices can be added in multiplication in the same way as normal indices.They should be left as improper fractions not mixed numbers. 117.Multiplying fractions: Put the product orf the numerators over the product of the denomiators and then simplify ifneeded.118.Multiplying matrices together: When you multiply two matrices together, each row in the first matrix combines with each column of the second to give a single number. (The first to the left will combine with the uppermost, the second to the left will combine with the second down)119.Multiplying with indices: Add indices, numbers stay120.n(A): Number of elements in set A121.Natural numbers: Whole, positive122.Negative indices: If something is raised to a negative indices, find the reciprocal.123.Obtuse cosine: The cosine of an obtuse angle is equal to the negative of its supplementary angle (cos100 = -cos80)124.Obtuse sine: The sine of an obtuse angle is equal to that of its supplementary angle (sin100 = sin80)125.Order of multiplied matrices: You can only multiply matrices if the number of columns in the first is equal to the number of rows in the second. The other two values willcombine to give the order of he product.126.Order of operations: BEDMAS127.Order of the matrix: The number of rows and columns in a matrix. Written as rows x columns128.Parallel lines on a graph: For a line to be parallel it must have the same gradient, so variable m must be the same inboth equations in y=mx + c 129.Parallelogram:Opposite sides are parallel and equal. Opposite angles areequal.130.Percentage change: change/original x 100131.Perpendicular lines on a graph: If a line is perpendicular to the other line, the gradient will be the negative reciprocal of it.Also, the product of both gradients will be 1132.Pictograms:Pictograms are frequency tables which use pictures to represent frequency. Each picture represents a number of items.It helps people to understand it more quickly but doesn'tallow for fractions of pictures.133.Pie charts:These do not show individual frequencies but instead compare the frequencies. Each is represented as a sector of the circle where the angle of each sector is proportional to the frequency it represents. The sectors should always be labelled. 134.pi x diameter: Circumference of a circle135.pi x radius squared: area of a circle136.Prime factorisation: Writing a number as a product of its prime factors (use a factor tree, until all bottom numbers are prime)137.Proper subset: All subsets except the original set (P)138.Quadratic graphs - parabolas: This will have either a u or upside down u shape and will be a smooth, continuous curve with no straight lines139.Quadratic sequences: Compare to square numbers first.Difference between the difference between each number (2nd difference) is the same. 1/2 of the 2nd difference will be thecoeffecient of n squared. Take that away from originalsequence and find the rule for the remaining numbers.140.Range: The range is the highest value in the set minus the lowest value in the set. It shows the spread of the data, it can help to compare data and consistency.141.Rates: Speed applied more generally to show changes in quantities. e.g. temperature goes down by 5 degrees everyminute142.Ratio: Ratio is a way of comparing the sizes of two or more quantities. and will be set out in the form x:y. It can also begiven in the form x/y. All the units in a ratio must be the same 143.Ratios when only some information is known: Find one part of the ratio. If 30 is 2 parts of the total ratio, divide it by 2 to find one part.144.Real numbers: Integers including decimals - two subsets 145.Real numbers - irrational: Decimals or numbers that cannot be written as a fraction146.Real numbers - rational: Integers or fractions147.Reciprocal graphs - y=a/x: Values should be rounded to two decimal points. x will never equal zero. It will besymmetrical. The graph never touches either axes.148.Reﬂecting a shape over an axis: The easiest and most effective methods is counting how many squares away each individual point is from the mirror line and then making thepoint that many squares away on the opposite side. Thisshould be done using the most direct route (even diagonally through squares)149.Relative frequency: Also known as experimental probability, it is given by frequency of the outcome of event/total number of trials. This relative frequency is an estimate of theprobability150.Reverse percentages - multiplier method: 110%=x. Find the original amount: x/1.1 (the multiplier) = the original amount.88%=x. Find the original amount: x/0.88 (the multiplier) = the original amount.151.Reverse percentages - unitary method: 110%=x. Find the original amount: 1%=x/110 original price = 1% x 100 (Used for decreases as well)152.Rhombus:All sides are equal, opposite sides are parallel. Diagonals bisect at right angles. Opposite angles are equal 153.Rotating a shape: The easiest and most effective way torotate a shape about a point is to count how many squaresacross and up and down each individual point is away from the axis, then turn your paper the amount required and count the squares using the new axes.154.Rotational symmetry: A 2D shape has rotational symmetry if it can be rotated about a point to look exactly the same in a new position. It can be represented by the order of rotational symmetry or number of times it can be rotated to look exactly the same until it is back to its original position. e.g. anequilateral triangle has a rotational symmetry of three155.Rotational symmetry of 3D shapes: 3D shapes have axes of symmetry. A cubiod has three axes of symmetry. Each axis has its own order of rotational symmetry.156.Scatter diagrams:Compares two variables by plotting corresponding values ona graph. They can have positive, negative or zero correlationbetween the points.157.Sector: Part of a circle bounded by two radii and one of the arcs.158.Similar shapes: Shapes are similar if one is an enlargement or reduction of the other. The corresponding angles of similar shapes are equal. Their sides can be found using ratios orlinear scale factors159.Simplifying algebraic fractions: Always fully factorise and cancel common brackets. Brackets that are the same withopposite sides are equal to -1 on the numerator. Two negatives over each other cancel each other out. Variables should be in brackets before they are cancelled.160.sine/cosine/tangent of known angle = side/side is used to: calculate the length of a side in a right-angledtriangle161.Sine rule notes: When you are calculating a side, use the rule with sides on top. When you are calculating an angle, use the rule with sines on top. Is not restricted to right angledtriangles162.Sketching an inequalities graph - linear programming: Sketch a broken line if the symbol is not equal to. Sketch asolid line if it is. Shade the unwanted region, e.g. if the wanted is y is less than x, shade the region where y is more than x. 163.Solve Direct proportion (a ∝ b): Divide to find the constant of proportionality (a=kb, k=constant)164.Solve Inverse proportion (a ∝ the reciprocal of b): Find total by multiplying values (a=k/b, k=constant)165.Solve x²+6x-7 by completing the square: (x+(6/2))^2 -(6/2)^2 - 7 = (x+3)^2 -3^2 - 7 = (x+3)^2 - 16.x=-2 +or- the square root of 16166.Solving problems of angles of elevation and depression: Use the trigonometric ratios(sine/cosine/tangent)167.Solving quadratic equations by completing the square: Solves quadratic equations by rewriting x squared + px +q in the form (x+a)^2 +b. Found by putting it into the form (x+(p/2))^2 -(p/2)^2 + q and rearranging to solve.168.Symmetry in circles - bisector of chords: The perpendicular bisector of a chord passes through the centre of the circle169.Symmetry in circles - chords: Two chords of equal length are the same distance from the centre of the circle.170.Symmetry in circles - tangents: Two tangents extending from a point to touch the circle at any two points will be the same length171.Symmetry of 3D shapes:3D shapes have planes of symmetry. One is a reflection of the other half. A cuboid has 3 planes of symmetry 172.Tangent: A tangent is a straight line that touches a circle or curve as one point only. It is drawn perpendicular to the radius 173.The determinant of a matrix: The determinant helps find the inverse. It can be represented by two lines around theletter representing the matrix. It is found using the formula ad -bc of matrix abcd 174.The identity matrix: A 2 x 2 matrix with 1 0 as its top numbers and 0 1 as its bottom numbers. This matrix is thesame as multiplying it by 1.175.The inverse sine/cosine/tangent of known side/known side is used to: Find the angles in a right-angled triangle 176.The magnitude of a vector: the square root of x square plus y squared - written with two vertical lines on either side of the vector177.Theoretical probability vs experimental probability: The way of calculating a probability is actually only theoreticaland in practice, this probability is only an estimate of the real results. However, the more times you take data, the closer the experimental probability will get to the theoretical probability 178.The probability of an event occurring =: 1 - the probability of the event not occurring.179.The probability scale: The probability scale goes from 0 being impossible to 1 being certain. Any probability values fall in between 0 and 1.180.the quadratic formula:181.The zero matrix (Z): The zero matrix is a 2 x 2 matrix which is just zeros and acts like a normal zero in equations.182.Three sides: Cosine rule followed by sine rule183.Transformations using matrices: You can transform a shape using matrices, the matrix for a shape is the vector for each point put together e.g. a triangle will form a 2 x 3 matrix.You can then multiply it by a specific 2 x 2 matrix to find the vectors of the transformed shape.184.Trapezium:Two parallel sides. The sum of the interior angles at the ends of each non-parallel side is 180 degrees.185.Tree diagrams: Probabilities of sequences of events can be shown on a tree diagram. Each brach represents an outcome which branches into more outcomes. When doing this type of diagram, whether the outcome is put back into the total should be taken into consideration.186.U angles on parallel lines:Called interior angles. They add up to 180 degrees187.Upper and lower bounds: Minimum and maximum any rounded number could possibly be188.Upper and lower bounds e.g. 27.5≥x>28.5: The upper bound is the maximum possible the rounded number couldhave been before rounding and the lower bound the opposite.Upper bound will be the number with 5 after the last decimal or as a unit depending on how it is rounded. The lower bound is the number 5 after one less than the last unit.ing coordinates - gradient: difference between y-coordinates/difference between x-coordinatesing coordinates - midpoints: Add end values and divide by two for each x and y separatelying coordinates -the distance between two points: Use pythagoras' thereom to find the distance by using thegradients as the sides of a right angled triangleing frequency tables to ﬁnd the mean: To find the mean, the frequency must be multiplied by the by the class it is in. These final values should then be added up and divided by the total frequency. If the classes are grouped, use themiddle value for the multiplication.ing frequency tables to ﬁnd the median: To find the median from a frequency table, add all the values to find the total number of cars surveyed then divide this by 2 to find the middle value. Now add the frequencies up cumulatively to find which group contains this middle number.ing rates: Temperature lowers 5 degrees every minute.After 6 minutes, temperature lowers (5x6=30) 30 degrees.Temperature lowers by 1 degree in (1/5=0.2) 0.2 minutes.Temperature lowers by 15 degrees in (15/5=3) 3 minutes 195.Vectors: Vectors have both a magnitude and a direction. They can be represented by the start and end points with an arrow over top or as a lower case letter printed in bold ORunderlined. On graphs they are represented by lines witharrows indicating direction196.Vectors on a coordinate grid: On a coordinate grid, vectors can be represented by two numbers in a bracket as a fraction without the line. The top number is the amount moved across (negative left) and the bottom number is the amountmoved vertically (negative down)197.Venn diagrams - A': Complement of A (all items not in A)198.Vulgar fraction: A fraction represented by a numerator and denominator and not by decimals.199.Vulgar fractions: All fractions excluding mixed fractions 200.Which ratio to use: SOHCAHTOA201.x to the power of -5: The reciprocal of x to the power of 5 (1/x to the 5)202.x to the power of ½: the square root of x203.x to the power of -¼: 1/the fourth root of x204.x to the power of ¾: (the fourth root of x)cubed205.x to the power of -¾: 1/(the fourth root of x)cubed 206.y=mx + c: m is the gradient of the line, c is where the line intercepts the y axis.207.Z angles on parallel lines:Called alternate angles. They are the same. 208.θ/360 x pi x diameter: Arc length209.θ/360 x pi x radius squared: Sector area210.θ (theta): the angle at the centre of a sector。

igcse数学词汇

igcse数学词汇代数部分1.基础在代数中，我们需要掌握一些基本的术语和符号，例如：add，plus 表示加法subtract 表示减法difference 表示差multiply，times 表示乘法product 表示积divide 表示除法divisible 表示可被整除的divided XXX 表示被整除dividend 表示被除数divisor 表示除数XXX 表示商remainder 表示余数factorial 表示阶乘power 表示乘方radical sign，root sign 表示根号round to 表示四舍五入to the nearest 表示四舍五入到最近的数2.有关集合在代数中，我们还需要了解一些集合的概念，例如：n 表示并集XXX 表示真子集XXX 表示解集3.有关代数式、方程和不等式在代数中，我们需要掌握一些代数式、方程和不等式的术语，例如：algebraic term 表示代数项like XXX，similar XXX 表示同类项4.基本数学概念在代数中，我们需要掌握一些基本的数学概念，例如：XXX 表示算术平均值XXX 表示加权平均值geometric mean 表示几何平均数XXX 表示指数，幂base 表示乘幂的底数、底边cube 表示立方数、立方体square root 表示平方根XXX root 表示立方根common XXX 表示常用对数digit 表示数字constant 表示常数variable 表示变量XXX 表示反函数XXX 表示余函数linear 表示一次的、线性的XXX 表示因式分解absolute value 表示绝对值round off 表示四舍五入5.有关数论在代数中，我们需要了解一些数论的概念，例如：natural number 表示自然数positive number 表示正数negative number 表示负数odd integer 表示奇整数odd number 表示奇数even integer，even number 表示偶数integer，whole number 表示整数6.有关分数和小数在代数中，我们需要了解一些分数和小数的概念，例如：XXX 表示真分数XXX 表示假分数mixed number 表示带分数vulgar n，common XXX 表示普通分数XXX 表示简分数XXX 表示繁分数numerator 表示分子XXX 表示分母least) common denominator 表示(最小)公分母XXX 表示四分之一Decimal ns are numbers that have no whole number component。