杨浦五角场初中补习班恒高一对一数学求两条直线间的距离
杨浦补习班五角场恒高教育英语初中阅读及作文专项训练

阅读&作文版块ReadingAThese days it is very common for young men to give flowers to young women when they are in love, but this hasn’t always been the custom.In the 1700s in Turkey, it was quite popular for people in love to secretly send each other baskets full of strange things. Usually, an old woman who sold flowers or fruits in the street left the basket beside the door of the person receiving it.These gift baskets included many kinds of objects, such as flowers, stones, feathers, wax, and even charcoal (木炭). Each thing in the basket had a special meaning and the person who received the basket could determine (测定) the true feelings of the giver.A bunch of flowers told young ladies about the feelings in the hearts of young men. Each different flower had a different meaning. For example, the flowers from an orange tree meant, “You are beautiful and pure.” Pink carnations meant, “My love for you is strong and great.” Yellow roses, on the other hand, meant, “I saw you with someone else.”Many flower dictionaries were made to help young people in love understand the meaning of the flowers they received. Not all of the dictionaries agreed, however, on the meaning of each flower, so a person had to watch out what flowers they chose to send. For example, depending on(依据) which dictionary you used, and which color you gave, a young man’s roses could mean, “I love you,” “love is dangerous,” or even, “my love has decreased(减少).”By the 1880s, using flowers to send messages had fallen out of fashion(流行), and the more direct way of sending love letters began. Today, flowers are still considered a lovely gift, but the meaning for each kind of flower has been lost.( ) 1. What custom is talked about in the second and third paragraphs?A. Sending gift baskets.B. Sending flowers.C. Selling gift baskets.D. Selling flowers.( ) 2. What did the items in a gift basket explain to the receiver?A. The receiver’s looks.B. The receiver’s personality.C. The sender’s feelings.D. The sender’s name.( ) 3. Where was the idea of sending gifts of love popular in 1700s?A. Asia.B. Turkey.C. Europe.D. North America.( ) 4. How did people know the meaning of each kind of flower?A. They found out from books.B. The sender told them.C. Old women explained it.D. They read secret letters.( ) 5. The word “decreased” in the passage means “______”.A. goes upB. goes downC. stays the sameD. varies( ) 6. What can be the best title of this passage?A.The introduction of sending flowers to send messagesB.How to send flowers.C.Sending flowers has fallen out of fashion.D.Different flowers have different meanings.B. Choose the words or expressions and complete the passage(选择最恰当的单词或词语完成短文)(12分)The impression you make at the beginning of an interview is very important. Interviewers often decide to hire someone in the first three minutes of the interview. They judge you by your appearance, attitude (态度) and manners.A friendly smile when you walk into the room is important. A smile shows a 80 and positive attitude. When you introduce yourself, look into the interviewer. Some interviewers offer a hand-shake, others don’t.Try to be as 81 as possible. But pay attention to your body language. The way you sit, walk, use your voice and show feeling on your face are all parts of your body language. It makes the interviewer know how you feel about yourself. Are you feeling positive about yourself? Your abilities? Your interest in the job?Speak clearly and loudly enough. show interest and enthusiasm(热情) in your 82 . When you speak, look at the interviewer. A lso don’t say negative things about yourself.Listen to questions carefully. 83 you don’t understand a question, ask the interviewer to repeat or explain."I’m sorry, but I didn’t catch that. I’m not sure exactly what you mean."Almost everyone is nervous in a job interview. Interviewers know that they don’t expect you to be completely 84 and relaxed. But they expect you to try to control your nervousness. They expect you to show confidence in your ability to do the job.At the end of the interview, 85 the interviewer for her or him. I t’s a good idea to senda short thank-you letter right after the interview, or deliver it by hand.80. A)confident B) dissatisfied C) unhappy D) excited81. A) smart B) nervous C) natural D) strict82. A) sound B) voice C) manner D) action83. A) Unless B) If C) Until D) As84. A) quiet B) silent C) calm D) still85. A) praise B) thank C) admire D) greetC. Read the passage and fill in the blanks with proper words(在短文的空格内填入适当的词,使其内容通顺,每空格限填一词,首字母已给))(14分)Wonder why you can get angry so easily while your friend smiles all the time? It’s p___86___ because you both have different personalities(性格) .Personality is also about how people think, behave, and react(反应) to e__87____ around them from day to day.So what makes people think and behave in certain ways?Part of the reason is that people are born like this. A baby gets its blood type, genes(基因)and other physical things when it’s still inside its mother. These things may help decide what the baby will be l__88____.But one’s personality doesn’t stop here. Family life, school learning and life e xperience can also make you the person you are. This doesn’t m ean it’s i__89____ to change your personality. You can alw ays try to make yourself better. Don’t get too worried about your weak points. Just a__90____ them. This is a good way to start making changes.If you don’t know how to make friends, find out why. Is it because you are too s___91___? Tell yourself to smile at people. Start talking to people using warm greetings.Don’t give yourself a hard time about it all. It’s not easy to change life h___92___ in one night. Keep working at it. One day you’ll see that you can turn over a new lea f and be a new one.D.Answer the questions(根据短文内容回答下列问题)(12分)If you go into the forest with friends, stay with them .If you don’t, you may get lost. If you do get lost, this is what you should do.Sit down and stay where you are. Don’t try to find your friends. Let them find you. You can help them to find you by staying in one place. That’s the most important thing you should do.There is another way to help your friends or other people to find you. Give them a signal(信号)outing or whistling three times. Stop. Then shout or whistle three times again. Any signal given three times is a call for help.Keep on shouting or whistling, always three times together. When people hear you, they will know that you are not just making noise for fun.They will let you know that they have heard your signal. they will give two shouts or two whistles. When a signal is given twice, it is an answer to a call for help.If you don’t think that you will get help before night comes, try to make a little house---cover up the hole with branches with lots of leaves. Make yourself a bed with leaves and grass.When you need some water, you have to leave your little branches house to look for a stream. Don’t just walk away. Pick off small branches and drop them as you walk in order to find the way back.93.When you go into forest with friends, what will you do?_________________________when I go into the forest.94.If you are lost in the forest, can you go to find them?________,________________________________.95.How can you let people believe that you are not just making noise for fun?I should____________________________________________________.96.When can you know there is an answer to a call for help?_______________________________, it is an answer to a call for help.97.When you don’t get help before night comes, what can you do?__________________________________________________________.98.What do you think the most important thing is when you are lost?__________________________________________________________.Writing:Write a passage of at least 60 words on the topic “The importance of reading newspapers”(以“阅读报纸的重要性”为题,写一篇不少于60个词的短文,标点符号不占格。
20-21版:2.2.4 点到直线的距离(步步高)

2.2.4 点到直线的距离 学习目标 1.了解点到直线的距离公式的推导方法.2.掌握点到直线距离的公式,并能灵活应用于求平行线间的距离等问题.3.初步掌握解析法研究几何问题的方法.知识点一 点到直线的距离1.定义:平面内点到直线的距离,等于过这个点作直线的垂线所得垂线段的长度. 2.图示:3.公式:d =|Ax 0+By 0+C |A 2+B 2. 知识点二 两条平行直线间的距离1.定义:两条平行线之间的距离等于其中一条直线上任意一点到另一条直线的距离.2.图示:3.求法:转化为点到直线的距离.4.公式:两条平行直线l 1:Ax +By +C 1=0与l 2:Ax +By +C 2=0之间的距离d =|C 2-C 1|A 2+B 2 .(A ,B 不全为0,C 1≠C 2)1.点P (x 0,y 0)到直线y =kx +b 的距离为|kx 0+b |1+k2.( × ) 2.直线外一点与直线上一点的距离的最小值是点到直线的距离.( √ )3.两平行线间的距离是一条直线上任意一点到另一条直线的距离,也可以看作是两条直线上各取一点的最短距离.( √ )4.直线x =x 1与直线x =x 2的距离为d =x 2-x 1.( × )一、点到直线的距离例1 (1)求点P (2,-3)到下列直线的距离.①y =43x +13;②3y =4;③x =3. 解 ①y =43x +13可化为4x -3y +1=0, 点P (2,-3)到该直线的距离为|4×2-3×(-3)+1|42+(-3)2=185. ②3y =4可化为3y -4=0,由点到直线的距离公式,得|-3×3-4|02+32=133. ③x =3可化为x -3=0,由点到直线的距离公式,得|2-3|1=1. (2)求过点M (-1,2),且与点A (2,3),B (-4,5)距离相等的直线l 的方程.解 方法一 当过点M (-1,2)的直线l 的斜率不存在时,直线l 的方程为x =-1,恰好与A (2,3),B (-4,5)两点的距离相等,故x =-1满足题意.当过点M (-1,2)的直线l 的斜率存在时,设l 的方程为y -2=k (x +1),即kx -y +k +2=0.由点A (2,3)与点B (-4,5)到直线l 的距离相等,得|2k -3+k +2|k 2+1=|-4k -5+k +2|k 2+1, 解得k =-13, 此时l 的方程为y -2=-13(x +1), 即x +3y -5=0.综上所述,直线l 的方程为x =-1或x +3y -5=0.方法二 由题意,得l ∥AB 或l 过AB 的中点,当l ∥AB 时,设直线AB 的斜率为k AB ,直线l 的斜率为k l ,则k l =k AB =5-3-4-2=-13, 此时直线l 的方程为y -2=-13(x +1), 即x +3y -5=0.当l 过AB 的中点(-1,4)时,直线l 的方程为x =-1.综上所述,直线l 的方程为x =-1或x +3y -5=0.反思感悟 (1)应用点到直线的距离公式时应注意的三个问题①直线方程应为一般式,若给出其他形式应化为一般式.②当点P 在直线l 上时,点到直线的距离为0,公式仍然适用.③直线方程Ax +By +C =0,当A =0或B =0时公式也成立,但由于直线是特殊直线(与坐标轴垂直),故也可用数形结合求解.(2)当用待定系数法求直线方程时,首先考虑斜率不存在是否满足题意.跟踪训练1 (1)若点P (3,a )到直线x +3y -4=0的距离为1,则a 的值为( ) A. 3B .-33 C.33或- 3 D.3或-33 答案 D解析 由题意得,|3+3a -4|1+(3)2=1, 即|3a -1|=2,解得a =3或-33. (2)已知坐标平面内两点A (3,2)和B (-1,4)到直线mx +y +3=0的距离相等,则实数m 的值为________.答案 -6或12解析 由|3m +2+3|m 2+1=|-m +4+3|m 2+1, 得|3m +5|=|m -7|,∴m =-6或m =12. 二、两平行线间的距离例2 (1)已知两平行直线l 1:3x +5y +1=0和l 2:6x +10y +5=0,则l 1与l 2间的距离为________.答案 33468解析 l 2:6x +10y +5=0可以化为3x +5y +52=0,∴l 1与l 2间的距离d =⎪⎪⎪⎪52-132+52=3234=33468. (2)已知直线l 与两直线l 1:2x -y +3=0和l 2:2x -y -1=0的距离相等,则l 的方程为________________.答案 2x -y +1=0解析 设直线l 的方程为2x -y +C =0,由题意,得|3-C |22+12=|C +1|22+12,解得C =1, ∴直线l 的方程为2x -y +1=0.反思感悟 求两平行线间的距离,一般是直接利用两平行线间的距离公式,当直线l 1:y =kx+b 1,l 2:y =kx +b 2,且b 1≠b 2时,d =|b 1-b 2|k 2+1;当直线l 1:Ax +By +C 1=0,l 2:Ax +By +C 2=0,且C 1≠C 2时,d =|C 1-C 2|A 2+B 2.但必须注意两直线方程中x ,y 的系数对应相等. 跟踪训练2 (1)两直线3x +y -3=0和6x +my -1=0平行,则它们之间的距离为______. 答案 104解析 由题意,得63=m 1≠-3-1,∴m =2, 将直线3x +y -3=0化为6x +2y -6=0,由两平行线间的距离公式,得|-1+6|62+22=540=104. (2)已知△ABC 的两顶点A ,B 在直线l 1:2x -y +3=0上,点C 在直线l 2:2x -y -1=0上,若△ABC 的面积为2,则AB 边的长为________.答案 5解析 点C 到AB 的距离即为l 1与l 2之间的距离,∴d =|-1-3|22+(-1)2=45=455, S △ABC =12|AB |·d =2, ∴|AB |=4÷455= 5. 三、距离公式的应用例3 (1)已知实数x ,y 满足6x +8y -1=0,则x 2+y 2-2y +1的最小值为________. 答案 710解析 ∵x 2+y 2-2y +1=(x -0)2+(y -1)2,∴上式可看成是一个动点M (x ,y )到定点N (0,1)的距离,即为点N 到直线l :6x +8y -1=0上任意一点M (x ,y )的距离,∴S =|MN |的最小值应为点N 到直线l 的距离,即|MN |min =d =|8-1|62+82=710. (2)两条互相平行的直线分别过点A (6,2),B (-3,-1),并且各自绕着点A ,B 旋转,如果两条平行直线间的距离为d .①求d 的取值范围;②求d 取最大值时,两条直线的方程.解 ①设经过点A 和点B 的直线分别为l 1,l 2,显然当⎩⎪⎨⎪⎧l 1⊥AB ,l 2⊥AB 时,l 1和l 2的距离最大, 且最大值为|AB |=(-3-6)2+(-1-2)2=310,∴d 的取值范围为(0,310].②由(1)知,d max =310,因为过点A ,B 直线的斜率为13, 所以d 取最大值时两平行线的斜率k =-3,两直线的方程分别为3x +y -20=0或3x +y +10=0.反思感悟 (1)(x -a )2+(y -b )2可表示为点(x ,y )与点(a ,b )之间的距离,将“数”转化为“形”,从而利用图形的直观性解决问题.(2)两平行线间的距离可转化为两点间的距离,通过两点间的距离利用数形结合思想得到两平行线间距离的最值.跟踪训练3 (1)已知P ,Q 分别是直线3x +4y -5=0与6x +8y +5=0上的动点,则|PQ |的最小值为( )A .3B. 3C.32D.32答案 D解析 两平行线间的距离就是|PQ |的最小值,3x +4y -5=0可化为6x +8y -10=0,则|PQ |=|5-(-10)|62+82=32. (2)动点P (x ,y )在直线x +y -4=0上,O 为原点,则|OP |最小时点P 的坐标为________. 答案 (2,2)解析 直线上的点到原点距离的最小值即为原点到直线的距离,此时OP 垂直于已知直线,则k OP =1,∴OP 所在的直线方程为y =x .由⎩⎪⎨⎪⎧ y =x ,x +y -4=0,解得⎩⎪⎨⎪⎧x =2,y =2. ∴点P 的坐标为(2,2).1.已知原点O (0,0),则点O 到直线x +y +2=0的距离等于( )A .1B .2 C. 2 D.22答案 C解析 d =|0+0+2|12+12= 2. 2.已知点(a ,1)到直线x -y +1=0的距离为1,则a 的值为( )A .1B .-1 C. 2 D .±2答案 D解析 由题意知,|a -1+1|12+12=1, 即|a |=2,∴a =±2.3.直线x -2y -1=0与直线x -2y -C =0的距离为25,则C 的值为( )A .9B .11或-9C .-11D .9或-11 答案 B解析 两平行线间的距离为d =|-1-(-C )|12+(-2)2=25, 解得C =-9或11.4.两平行直线3x +4y +5=0与6x +ay +30=0间的距离为d ,则a +d =________. 答案 10解析 由两直线平行知,a =8,d =|15-5|5=2, ∴a +d =10.5.直线3x -4y -27=0上到点P (2,1)距离最近的点的坐标是________________. 答案 (5,-3)解析 由题意知过点P 作直线3x -4y -27=0的垂线,设垂足为M (图略),则|MP |为最小距离,直线MP 的方程为y -1=-43(x -2), 解方程组⎩⎪⎨⎪⎧ 3x -4y -27=0,y -1=-43(x -2),得⎩⎪⎨⎪⎧x =5,y =-3 ∴所求点的坐标为(5,-3).1.知识清单:(1)点到直线的距离.(2)两平行线之间的距离.2.方法归纳:公式法、分类讨论、数形结合.3.常见误区:求两条平行线之间的距离时,只需把两直线方程化成一般式,且x 与y 的系数对应相同.。
两条直线的交点坐标、两点间的距离 课件

一、预习教材·问题导入 根据以下提纲,回答下列问题: (1)直线上的点与其方程 Ax +By+C=0 的解有什么样的关 系?提示:直线l上每一个点的坐标都满足直线方程,也就是说
直线上的点的坐标是其方程的解.反之直线l的方程的每一 个解都表示直线上的点的坐标.
由方程组y8=x+3,6y=25,
解得x=78, y=3,
由于反射光线为射线,
故反射光线的方程为y=3x≤78. 由光的性质可知,光线从O到P的4-(-4)=8, ∴光线从O经直线l反射后到达P点所走过的路程为8.
[类题通法]
二、归纳总结·核心必记 1.两条直线的交点坐标
(1)求法:两个直线方程联立组成方程组,此方程组的解 就是这两条直线的交点坐标,因此解方程组即可. (2)应用:可以利用两条直线的 交点个数 判断两条直线的 位置关系.
一般地,直线l1:A1x+B1y+C1=0和直线l2:A2x+B2y+ C2=0的位置关系如表所示:
[解] 设原点关于l的对称点A的坐标为(a,b),由直线 OA与l垂直和线段AO的中点在l上得
ba·-43=-1, 8×a2+6×b2=25,
解得ab= =43, ,
∴A的坐标为(4,3).
∵反射光线的反向延长线过A(4,3),
又由反射光线过P(-4,3),两点纵坐标相等.
故反射光线所在直线方程为y=3.
三、综合迁移·深化思维
两点P1(x1,y1),P2(x2,y2)间的距离公式是否可以写成|P1P2| = x1-x22+y1-y22的形式?
提示:可以,原因是
x2-x12+y2-y12 =
x1-x22+y1-y22 ,也就是说公式中P1,P2两点 的位置没有先后之分.
点到直线的距离 两条平行直线间的距离 课件

距离公式的综合应用
已知直线 l 过点 A(2,4),且被平行直线 l1:x-y+1 =0 与 l2:x-y-1=0 所截的线段中点 M 在直线 x+y-3=0 上,求直线 l 的方程.
【思路探究】 可设出点 M 的坐标,利用点 M 到两直线 的距离相等,求出点 M 的坐标,再用两点式写出直线的方程, 也可先求出与 l1,l2 平行且等距离的直线方程,再与 x+y-3 =0 联立求出 M 点的坐标,再由两点式写出直线方程.
【自主解答】 法一 ∵点 M 在直线 x+y-3=0 上,
∴设点 M 坐标为(t,3-t),则点 M 到 l1,l2 的距离相等, 即
|t-3-t+1|=|t-3-t-1|,
2
2
解得 t=32,∴M32,32. 又 l 过点 A(2,4),
由两点式得4y--3232=2x--3232, 即 5x-y-6=0,故直线 l 的方程为 5x-y-6=0.
②当 B=0,A≠0 时,直线 l 的方程为 Ax+C=0,x=- CA,d=x0+CA=|Ax|0A+| C|,适合公式;
③当点 P 在直线 l 上时,有 Ax0+By0+C=0, d=|Ax0+A2B+y0B+2 C|=0,适合公式.
点到直线的距离
(1)概念:过一点向直线作垂线,则该点与垂足 之间的距
1.应用距离公式解答有关问题时,要注意以下几点: (1)直线的方程是一般式,在用两平行线间的距离公式时, 两方程中 x,y 的系数分别相等; (2)要结合图形,帮助解答; (3)求直线方程时,要特别注意斜率不存在的情况.
2.常见的距离公式应用问题的解题策略 (1)最值问题: ①利用对称转化为两点之间的距离问题. ②利用所求式子的几何意义转化为点到直线的距离. ③利用距离公式将问题转化为一元二次函数的最值问 题,通过配方求最值.
两条直线的位置关系与距离公式-高考数学复习课件

x +4 y -7=0的直线的方程为 4 x -3 y +9=0
5
,
3
= −
2 + 3+1 = 0,
法一:由ቊ
解得൞
7
− 3 + 4 = 0,
= ,
9
故交点的坐标为
5
7
− ,
3
9
.
.
4
因为所求直线与直线3 x +4 y -7=0垂直,所以所求直线的斜率为 ,所
3
7
4
以所求直线的方程为 y - =
13
2
方法总结
距离问题的常见题型及解题策略
1. 求两点间的距离.关键是确定两点的坐标,然后代入公式即可,一般
用来判断三角形的形状等.
2. 解决与点到直线的距离有关的问题.应熟记点到直线的距离公式,若
已知点到直线的距离求直线方程,一般考虑待定斜率法,此时必须讨
论斜率是否存在.
3. 求两条平行线间的距离.要先将直线方程中 x , y 的对应项系数转化成
1
直
k 1·k 2=-
,当一条直线斜率为零,另一条直线斜率不存在时,两条直线
.
垂
2. 两直线相交
直线 l 1: A 1 x + B 1 y + C 1=0和 l 2: A 2 x + B 2 y + C 2=0的公共点的坐标
1 +1 +1 = 0,
与方程组ቊ
的解一一对应.
2 +2 +2 = 0
6. (2024·广东广州模拟)已知点 P (4, a )到直线4 x -3 y -1=0的距离不大
于3,则 a 的取值范围为
点 P 到直线的距离为
由
两直线间的距离公式是什么

两直线间的距离公式是什么假设我们有两条平行直线,分别表示为l1和l2、我们希望计算这两条直线之间的距离。
我们可以做以下步骤:1.找到l1和l2上的两个点P1和P22.画一条从P1到P2的向量V13.画一条从l1上的任意一点P到l2上的任意一点Q的向量V24.如果V1和V2是相互垂直的,则l1和l2之间的距离就是V2的长度。
为了更好地理解这个距离公式,我们可以尝试推导一个简单的例子。
假设我们有两条平行直线,l1和l2,它们之间的距离D是未知的。
我们可以选择l1上的两个点P1和P2,并且l2和l1之间的交点为Q。
通过连接P1和P2,我们得到了一个表示向量V1的线段。
同样,连接P到Q,我们得到了一个表示向量V2的线段。
由于l1和l2是平行的,我们可以确定V1和V2是相互垂直的。
那么,我们如何计算这个垂直距离呢?我们可以使用向量的点乘和向量的叉乘来计算。
首先,我们需要确定向量V1和向量V2的长度。
我们可以使用两点之间的距离公式来计算V1的长度。
假设P1的坐标为(x1,y1),P2的坐标为(x2,y2),根据两点之间的距离公式,我们有:V1 = sqrt((x2 - x1)^2 + (y2 - y1)^2)接下来,我们需要确定向量V2的长度。
由于V1和V2是相互垂直的,我们可以使用向量的点乘来计算它们之间的关系。
向量的点乘公式为:V1 · V2 = ,V1, * ,V2,* cos(θ)其中,θ为V1和V2之间的夹角。
由于V1和V2是相互垂直的,cos(θ)为0。
因此,点乘公式可以简化为:V1·V2=0将两个向量展开为坐标形式,我们可以得到:(x2-x1)*(x-x1)+(y2-y1)*(y-y1)=0将l2的方程式代入上述方程,我们可以得到:(x2-x1)*(x-x1)+(y2-y1)*[(x2-x1)/(y2-y1)]*(y-y1)=0化简上述方程,我们可以得到:(x-x1)*(x2-x1)+(y-y1)*(y2-y1)=0这个方程就是直线到点的距离公式。
杨浦五角场初中补习班恒高一对一数学求证线段是否垂直
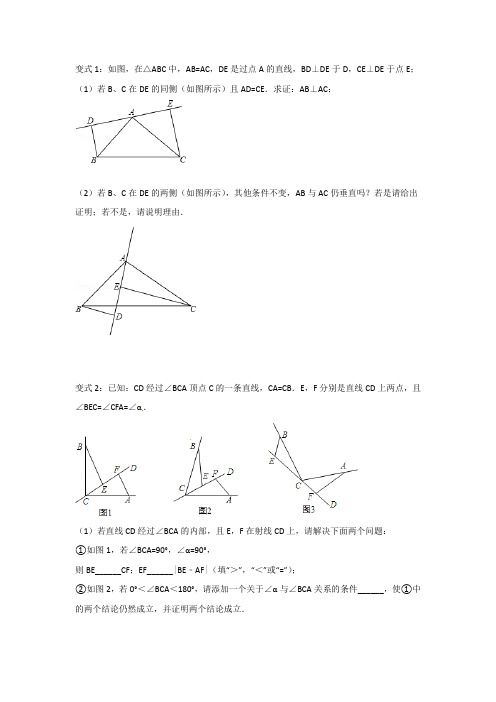
变式1:如图,在△ABC中,AB=AC,DE是过点A的直线,BD⊥DE于D,CE⊥DE于点E;(1)若B、C在DE的同侧(如图所示)且AD=CE.求证:AB⊥AC;
(2)若B、C在DE的两侧(如图所示),其他条件不变,AB与AC仍垂直吗?若是请给出证明;若不是,请说明理由.
变式2:已知:CD经过∠BCA顶点C的一条直线,CA=CB.E,F分别是直线CD上两点,且∠BEC=∠CFA=∠α.
(1)若直线CD经过∠BCA的内部,且E,F在射线CD上,请解决下面两个问题:
①如图1,若∠BCA=90°,∠α=90°,
则BE______CF;EF______|BE﹣AF|(填“>”,“<”或“=”);
②如图2,若0°<∠BCA<180°,请添加一个关于∠α与∠BCA关系的条件______,使①中的两个结论仍然成立,并证明两个结论成立.
(2)如图3,若直线CD经过∠BCA的外部,∠α=∠BCA,请提出EF,BE,AF三条线段数量关系的合理猜想(不要求证明).。
高中数学《第三章直线与方程3.3直线的交点坐标与距离公式3.3.4两条平行直线间的距离》88PPT课件

3、利用点到直线的距离公式求解。
求下列两条平行直线间的距离:
(1)x+2y-2=0
x+2y+4=0
|12 20 4 | 6 5
d
12 22
5
(2)两平行线3x-2y-1=0和6x-4y+2=0的距离是____.
应用新知
两条平行直线间的距离:
例2、求证:两条平行线l1:Ax+By+C1=0与
高中数学必修二
3.3.4 两条平行线间的距离
仪陇县第二中学 余浪
两条平行直线间的距离:
定义:两条平行直线 间的距离是指夹在两 条平行直线间的公垂 线段的长。
A l1
C
l2 B
D
结论:平行线间的距离处处相等。
两条平行直线间的距离: 探究新知
提问:l1与l2平行吗? 为什么?
l1的斜率为k1
2 7
l2的斜率为k2
2 7
两平行线间的
距离处处相等
yl1:2x-7y+14=0
A C
B
D
o
x
l2: 4x-14y-21=0
两条平行直线间的距离:
1、在l2上任取一
点,例如M(
21 4
,0)
yl1:2x-7y+14=0
2、M到l1的距离 等于l1与l2的距离
M
o
x
l2: 4x-14y-21=0
平行直线间的距离转化为点到直线的距离
两条平行直线间的距离:yl1:2x-7y+14=0
解:取l2与x轴的交
点M,则M(
21 4
,0)
M
o
x
两个平行线段之间的间隔

两个平行线段之间的间隔1. 垂直距离法如果我们想要计算两个平行线段的最短垂直距离,可以使用垂直距离法。
该方法通过将两个线段的起始点分别垂直投影到另一个线段上,然后计算投影点之间的距离来确定最短垂直距离。
具体步骤如下:1. 找到第一个线段的起始点和结束点,记作(A₁, A₂);2. 确定第二个线段上与第一个线段起始点垂直的点(B₁)和与第一个线段结束点垂直的点(B₂);3. 计算点(A₁, A₂)到点(B₁, B₂)之间的距离,即为两个平行线段之间的最短垂直距离。
2. 并行直线法另一种计算两个平行线段间隔的方法是使用并行直线法。
这种方法利用平行线的特性,即两个平行线上的任意一条垂线都与另一个线上的所有点都垂直。
具体步骤如下:1. 找到第一个线段的起始点和结束点,记作(A₁, A₂);2. 找到第二个线段上与第一个线段起始点对应的点(B₁);3. 由于两个线段平行,所以线段(B₁, A₁)也与第一个线段平行;4. 计算线段(B₁, A₁)的长度,即为两个平行线段之间的间隔。
3. 数学公式法除了几何方法外,还可通过数学公式来计算两个平行线段之间的间隔。
这种方法适用于已知两个线段的具体坐标的情况。
具体步骤如下:1. 找到第一个线段的起始点和结束点,记作(A₁, A₂),其坐标分别为(x₁, y₁)和(x₂, y₂);2. 找到第二个线段的起始点和结束点,记作(B₁, B₂),其坐标分别为(x₃, y₃)和(x₄, y₄);3. 使用下面的数学公式计算两个平行线段之间的间隔:间隔 = |[(x₂ - x₁)(y₃ - y₄) - (x₃ - x₄)(y₂ - y₁)] / sqrt((x₂ -x₁)² + (y₂ - y₁)²)|结论本文介绍了计算两个平行线段之间间隔的三种方法:垂直距离法、并行直线法和数学公式法。
根据实际情况和所需精度,选择合适的方法进行计算。
在解决几何问题或进行相关分析时,这些方法将对我们提供帮助。
两直线的位置关系及距离公式

06
总结回顾与拓展延伸
总结回顾本次课程重点内容
两直线平行与重合的判定
两直线垂直的判定
点到直线的距离公式
两平行线间的距离公式
通过比较两直线的斜率,可以 判断两直线是否平行或重合。 若两直线斜率相等且不重合, 则两直线平行;若两直线斜率 相等且重合,则两直线重合。
两直线垂直的充分必要条件是 它们的斜率互为负倒数。即, 若一直线的斜率为m,另一直线 的斜率为-1/m,则这两直线垂 直。
通过比较两直线的斜率,若斜率 相等且截距不等,则两直线平行。
相交关系
定义
两直线在同一平面内,且斜率不相等,则称两直线相 交。
性质
相交直线有且仅有一个交点,且相交形成的角的大小 与两直线的斜率有关。
判定方法
通过比较两直线的斜率,若斜率不相等,则两直线相 交。
重合关系
定义
两直线在同一平面内,且斜率和截距都相等,则称两直线重合。
THANKS
感谢观看
在同一平面内,两条直线的位 置关系有平行、相交和重合三 种。
直线的倾斜角是直线与x轴正方 向之间的夹角,取值范围是[0,π)。
直线的方程形式
一般式
Ax + By + C = 0(A、B不 同时为0)。
斜截式
y = kx + b(k是斜率,b是 截距)。
点斜式
两点式
y - y1 = k(x - x1)(k是斜率, (x1, y1)三个顶点分别为$A(1, 2)$,$B(-3, -2)$, $C(5, 6)$,求三角形ABC的面积。
解析
首先求出三角形ABC的三边所在直线的方程,然后利用点 到直线的距离公式求出三角形的高,最后利用底和高求出 三角形的面积。
点到直线的距离、两条平行直线间的距离 课件

正解:当直线 l 过点 A(1,2)且斜率不存在时,直线 l 的方程为 x=1,原点到直线 l 的距离为 1,满足题意.
当直线 l 过点 A(1,2)且斜率存在时,由题意设直线 l 的方程为 y-2=k(x-1),即 kx-y-k+2=0.因为原点到直线 l 的距离为 1, 所以|-kk2++21|=1,解得 k=34.所以所求直线 l 的方程为 y-2=34(x- 1),即 3x-4y+5=0.
综上所述,所求直线 l 的方程为 x=1 或 3x-4y+5=0.
纠错心得:当用待定系数法确定直线的斜率时,一定要对斜率 是否存在进行讨论,否则容易犯解析不全的错误.
课堂总结
1.求点到直线的距离时,应先将直线的方程化成一般式,并 要注意公式的分子中含有绝对值.
2.点 P(x0,y0)到直线 x=a 的距离为 d=|x0-a|,到直线 y=b 的距离为 d=|y0-b|.
(3)当两直线都与 x 轴(或 y 轴)垂直时,可利用数形结合来解决.
典例剖析 题型一 点到直线的距离 【例 1】 求点 P0(-1,2)到下列直线的距离: (1)2x+y-10=0;(2)x=2;(3)y-1=0.
思路点拨:利用点到直线的距离公式,对于特殊直线也可数形 结合.
解: (1)由点到直线的距离公式知 d=|2×-212++122-10|=105=2 5. (2)法一:直线方程化为一般式为 x-2=0. 由点到直线的距离公式 d=|-1+102+×022-2|=3. 法二:∵直线 x=2 与 y 轴平行, ∴由图(1)知 d=|-1-2|=3.
6--3 故所求的直线方程分别为 y-2=-3(x-6)和 y+1=-3(x+ 3),即 3x+y-20=0 和 3x+y+10=0.
浦东一对一数学辅导 直线方程的总结

浦东一对一数学辅导 恒高1对1 直线方程的总结1.直线方程的几种形式1)点方向式(方向向量):00x x y y u v--=(0uv ≠); 2)点法向式(法向量):00()()0a x x b y y -+-=(,a b 不全为零); 3)点斜式:)(11x x k y y -=-(k 存在); 4)斜截式:b kx y +=(k 存在);5)截距式:1=+b ya x (0,0≠≠b a );6)两点式:121121x x x x y y y y --=--(2121,y y x x ≠≠); 7)一般形式(与其他形式的互化):0ax by c ++=(,a b 不全为零).2.直线的斜率、倾斜角(转化) 1)已知倾斜角α求斜率k2tan ,2a k a a ππ⎧=⎪⎪=⎨⎪≠⎪⎩不存在, ,当02a π≤<时,0k ≥;当2a ππ<<时,0k <.2)已知斜率k ,求倾斜角α,arctan ,0arctan ,0k k a k k π≥⎧=⎨+<⎩,3.两直线的位置关系1)直线的位置关系(可根据行列式、向量、方程判定)▲设直线12,l l 的方程分别为: 1111:0l a x b y c ++= ; 2222:0l a x b y c ++=它们的位置关系取决于方程组1112220a x b y c a x b y c ++=⎧⎨++=⎩的解的情况,可借助于行列式对方程组解加以讨论,也可直接利用两方程对应项系数之间的关系进行讨论.① 当方程组有无数组解时,两直线重合;② 当方程组有唯一解时,两直线相交,交点坐标即为方程组对应的一组解; ③ 当方程组无解时,两直线互相平行.▲① 已知直线1l 、2l 的方程为1l :0111=++C y B x A ,2l :0222=++C y B x A ,)0,0(222111≠≠C B A C B A ,则1l ∥2l 的充要条件是212121C C B B A A ≠= . ② 已知直线1l 和2l 的一般式方程为1l :0111=++C y B x A ,2l :0222=++C y B x A ,则1l ⊥2l 的充要条件是02121=+B B A A . 2)两直线的夹角① 设两条直线的方程为1111:0l a x b y c ++=(11,a b 不同时为零),2222:0l a x b y c ++=(22,a b 不同时为零),两直线的夹角为α,则cos α=② 设两条直线的方程分别为11112222:(),:()l y y k x x l y y k x x -=--=-,两条直线的夹角为α,则1212tan 1k k k k α-=+12(10)k k +≠.4.点与直线的关系 1)点到直线的距离设00(,)P x y ,直线方程为:0l ax by c ++=(,a b 不同时为零),则P 到直线l的距离d =2)两平行线间的距离设两平行直线的方程为11:0l ax by c ++=,22:0l ax by c ++=,则d =3)点与直线的位置关系(同侧、异侧,用0,0δδ><判定)已知点00(,)Q x y 和直线l :0ax by c ++=,令δ=则δ的值就可以是正的或是负的,当然也可以是零.① 当0δ=时表示点00(,)x y 在直线l :0ax by c ++=上; ② 对于使0δ>的所有点00(,)x y ,它们必位于直线l 的同一侧; ③ 对于使0δ<的所有点00(,)x y ,它们比位于直线l 的另一侧.这就是说,直线l 把平面分成三部分,分别为上述三部分。
杨浦恒高五角场一对一补习班2017学年第一学期高三联合调研

2017学年第一学期高三联合调研II. Grammar and VocabularySection A (20%)How to Find Happiness without Buying it?Our materialistic society has led us to believe that happiness cannot be obtained without having money.Rather than learning to be satisfied with what we have, we ___21___ (teach) to want more. We learn from advertising, and from the media, __22____ we need to buy trinkets and toys in order to make ourselves happy, or __23___ (fulfill) emotional needs, and that the purchases they are trying to talk us into will provide us with the psychological comfort we are looking for.Unfortunately, as a society we have bought into these ___24___ (misguide) messages and have come to believe that ___25__ (spend) money on certain items will bring us fame, fortune, happiness, beauty, or popularity. As a result, we trade hours of our lives working, sacrificing time that __26___ have been spent with our families, ___27__ the pursuit of the almighty dollar.Actually, there are a number of ways to enjoy life without the need for a great deal of money. For example, think about __28__ you would spend your time, and what you would do for enjoyment. Change your focus from material possessions to ___29__ that bring you enjoyment, such as spending quality time with your family and friends. Certainly, it is important to work and earn enough to provide for our basic needs and the needs of our families, but it is important to recognize when the desire for personal possessions becomes overly consuming, __30___ otherwise will upset a balance between a satisfying work life and a rich home life. The best way to achieve such a balance is to ensure the drive for material possessions does not become all consuming.A pen has always been a(n) (31) ________tool when it comes to taking exams. However, in this digital age, this traditional tool may become a thing of the past.Cambridge University in Britain is considering ending 800 years of written exams and allowing students to use laptops(手提电脑) or iPads to take exams instead.The move comes after Cambridge teachers complained that students’ handwriting is becoming too hard to recognize. The (32)______ in being able to write neatly could largely be down to the (33)______ on laptops in lectures and elsewhere.“There has definitely been a (34)______ trend. It is difficult for both the students and the examiners as it is harder and harder to read these scripts,” Sarah Pearsall, a senior lecturer at the university, told The Telegraph.As a result, more and more students with poor handwriting are being (35)______ to return to university during the summer holidays to read their answers aloud to university administrators.However, some (36)______ the move, fearing the handwritten word would become a lost art. Tracey Trussell, a handwriting expert at the British Institute of Graphologists, (37)______Cambridge to “make sure that students continue to write by hand, particularly in lectures”. She told The Telegraph that writing by hand could help students improve their memory and understand lessons better.Meanwhile, there are also concerns that primary and secondary schools could follow Cambridge’s examples.In fact, a similar plan was carried out for some first- and second-year students at Edinburgh University in the UK in 2011, reported The Scotsman. Senior officials at the university believed it was (38)______ to expect students to use pens and paper during exams when most of their coursework was done using computers.The move also echoes the opinions of Harvard professor Eric Mazur, known as the father of the “flipped classroom (翻转课堂)”. He believed that the rise of the (39)______ to mobile internet means that we live in an age in which we don’t need to memorize anything. Students should be tested on their creative and (40)______ skills, rather than the ability to remember information, he said during the Times Higher Education World Academic Summit in September.III. Grammar and VocabularySection AIt's no secret that as a population, Americans have been getting heavier, but researchers now say that our weight problem may be worse than we thought.In a study published in the journal PLOS One, lead author Dr. Eric Braverman says that our current measure of obesity (肥胖症) -- body mass index, or BMI -- significantly (41) ______ the number of people, especially women, who are obese.Braverman and his co-author, Dr. Nirav Shah, studied 1,400 men and women, comparing their BMI measurement to their percentage of body fat, as measured by a dual-energy X-ray absorptiometry (DEXA) scan. While BMI is a simple ratio of a person’s height and weight, the DEXA scan-- which is normally used to measure body density (密度) -- can (42) ______ between bone, fat and muscle mass.Based on BMI, about one-third of Americans are considered obese, but when other methods of measuring obesity are used, that number may be (43) ______ to 60% according to Braverman.Physicians have complained for years that BMI is a(n) (44) ______ measure of healthy weight. Extremely muscular people, (45) ______ , may weigh “too much” for their height, since dense muscle mass weighs more than fat, thus qualifying as obese even if their bodies contain very little fat. Yet it’s not extra weight itself, but excess fat that (46) ______ health problems.“People aren’t being diagnosed as obese, so they’re not being told about their risk of disease or being given (47) _____ on how to improve their health,” Braverman said. Data show that people who (48) ______ to put on pounds are more likely to continue getting heavier, increasing their risk for a number of diseases.So why is BMI still being used, if it’s not (49) ______? For now, it’s the best and easiest way for physicians to measure a person’s healthy weight while taking into account his or her general body (50) ______. DEXA scans are far too expensive to be used as a (51) ______ measure during doctor’s visits. BMI isn’t perfect, but many experts say it’s the best they have.Still, as results like Braverman’s continue to (52) ______, it may be time to consider other ways of tracking weight, and (53) ______, body fat. “It’s important to point out the (54) ______ of the BMI,” Dr.Richard Bergman, director of Cedars Sinai’s Obesity and Diabetes ResearchInstitute in Los Angeles said. “It’s a poor measure of (55) ______, and we do need better measures.”41、A. predicts B. underestimates C. increases D. reduces42、A. compare B. recognize C. identify D. distinguish43、A. closer B. related C. devoted D. key44、A. important B. imperfect C. incredible D. uncertain45、A. for example B. in contrast C. in addition D. without doubt46、A. arises from B. owes to C. leads to D. goes through47、A. views B. orders C. instruction D. focus48、A. decide B. start C. hope D. fear49、A. right B. recognizable C. popular D. precise50、A. structure B. well-being C. function D. weight51、A. special B. routine C. scientific D. decisive52、A. fill in B. run out C. go down D. build up53、A. in particular B. on occasion C. after all D. in all54、A. mistakes B. failure C. inferiority D. weakness55、A. health B. body C. fatness D. diseasesSection B(22分)(A)While a female politician or first lady can use her clothing to create a favorable public image, male politicians don’t have as many fashion choices to play with. But Canadian Prime Minister Justin Trudeau seems to have discovered a goldmine – his socks.He’s been seen wearing “statement socks” at many public events. For example, during a NATO(北大西洋公约组织) meeting in Brussels in May, Trudeau wore one blue sock and one pink, both with the NATO symbol printed on them. And in June, he wore a pair of socks with the pattern of maple leaves – a national symbol of Canada – when he appeared on a TV show.The New York Times’ editor Vanessa Friedman praised Trudeau’s play on his socks as “clever”. “Socks are subtle(微妙的) enough not to be distracting, but visible enough that you can’t miss the point,” she wrote. “Rarely have a man’s ankles said so much.” But not all the playful socks that Trudeau wears send some kind of political message – some of them are just for fun.One example is a pair he wore during a meeting with Enda Kenny, then the prime minister of Ireland, in Montreal on May 4. During their meeting, Trudeau wore Star Wars socks – simply because that day happened to be International Star Wars Day. If this pair of socks sent out any message, according to V ogue magazine reporter Emily Farra, that message was “just that he has a quirky side and loves George Lucas films,” she wrote.However, some say that 45-year-old Trudeau’s unusual sock choices show a childish side of the prime minister that proves he isn’t mature enough to be the leader of a country.But Friedman doesn’t see it that way. “The socks have been a source of pride and applause on an international scale – a symbol both of Mr Trudeau’s ability to embrace multiculturalism and of his position as a next-generation leader not bound by old traditions,” she wrote. “They have opened up possibilities for the future.”1.Trudeau wore a pair of socks with the pattern of maple leaves to _______A.distract audience’s attention from the TV showsB.send a message that he is proud of his countryC.narrow the gap between politician and civiliansD.claim that he is the new-generation leader of the country2.The underlined word “quirky” is closest in meaning to _______A.abnormalB.seriousC.enthusiasticD.odd3.According to Friedman, Justin Trudeau _______A.has a promising future but still an inexperienced politicianed a clever way to explore and finally discovered a goldmineC.is open-minded and is ready to accept different culturesD.is a leader who has abandoned old traditions to build up his future4.The article is mainly about _______A.the strange hobby of a young politicianB. a young leader’s clever choice of socksC.how male politicians maintain public imageD.the gender difference in modern politicians。
两直线间的距离公式是什么

两直线间的距离公式是什么在数学课堂学习中,我们会学到两直线间的距离公式,那么两直线间的距离公式是什么呢。
以下是由编辑为大家整理的“两直线间的距离公式是什么”,仅供参考,欢迎大家阅读。
两直线间的距离公式两平行线之间的距离公式:d=|C1-C2|/√(A²+B²)。
两平行线方程分别是:Ax+By+C1=0和Ax+By+C2=0。
两平行线之间的距离公式设两条直线方程为Ax+By+C1=0Ax+By+C2=0则其距离公式为|C1-C2|/√(A²+B²)推导:两平行直线间的距离就是从一条直线上任一点到另一条直线的距离,设点P(a,b)在直线Ax+By+C1=0上,则满足Aa+Bb+C1=0,即Aa+Bb=-C1,由点到直线距离公式,P到直线Ax+By+C2=0距离为d=|Aa+Bb+C2|/√(A²+B²)=|-C1+C2|/√(A²+B²)=|C1-C2|/√(A²+B²)学习数学的方法一)、课内重视听讲,课后及时复习。
新知识的接受,数学能力的培养主要在课堂上进行,所以要特点重视课内的学习效率,寻求正确的学习方法。
上课时要紧跟老师的思路,积极展开思维预测下面的步骤,比较自己的解题思路与教师所讲有哪些不同。
特别要抓住基础知识和基本技能的学习,课后要及时复习不留疑点。
首先要在做各种习题之前将老师所讲的知识点回忆一遍,正确掌握各类公式的推理过程,应尽量回忆而不采用不清楚立即翻书之举。
认真独立完成作业,勤于思考,从某种意义上讲,应不造成不懂即问的学习作风,对于有些题目由于自己的思路不清,一时难以解出,应让自己冷静下来认真分析题目,尽量自己解决。
在每个阶段的学习中要进行整理和归纳总结,把知识的点、线、面结合起来交织成知识网络,纳入自己的知识体系。
二)、适当多做题,养成良好的解题习惯。
要想学好数学,多做题是难免的,熟悉掌握各种题型的解题思路。
2024版高考数学总复习:两直线的位置关系距离公式课件

考点1
两直线平行与垂直判定及应用——基础性
1.“m=1”是“直线l1:mx+y-1=0和直线l2:x+my+6=0平行”
的(
)
A.充分不必要条件
B.必要不充分条件
C.充要条件
D.既不充分也不必要条件
A
解析:直线l1:mx+y-1=0和直线l2:x+my+6=0平行⇔m2=
l1∥l2
l1⊥l2
A1A2+B1B2=0
______________
l1与l2重合
特别地,若A2,B2,C2中存在为0的情况,则利用斜率关系判断.
(3)两直线相交
交点——直线l1 :A1x+B1y+C1 =0和l2:A2x+B2y+C2 =0的公共点
1 + 1 + 1 = 0,
的坐标与方程组ቊ
2 + 2
(3)两条平行直线Ax+By+C1=0与Ax+By+C2=0(其中A2+B2≠0,
1 − 2
2 + 2
C1≠C2)间的距离d=________________.
应用点到直线的距离公式和两平行线间的距离公式时应注意:
(1)将方程化为最简的一般形式.
(2)利用两平行线之间的距离公式时,应使两直线方程中x,y的系数
0 +
1+ 2
.
(4)两平行直线2x-y+1=0,4x-2y+1=0间的距离为0.
1
2
3
4
5
( × )
( × )
2.已知过点A(-2,m)和B(m,4)的直线与直线2x+y-1=0平行,
则实数m的值为(
A.0
B
)
B.-8
C.2
原创2:2.3.4 两条直线平行间的距离

综上所述,所求直线l1,l2的方程为 l1:x=0,l2:x=5或l1:12x-5y+5=0, l2:12x-5y-60=0.
题型方法
求两平行直线线距离求法
(1)把直线方程化为直线的一般式方程; (2)两条直线方程中x,y的系数必须分别相等; (3)两平行线间的距离是一条直线上任一点到另一条直线的 距离,也可以看作是两条直线上各取一点的最短距离.
d
| C1 C2 | A2 B2
.
3.求距离的最值要注意几何意义使用.
跟踪练习
1.若直线l1:x+my+6=0与l2:(m-2)x+3y+2m=0互相平行,则m的值为
间的距离为
.
,它们之
解析:由m(m-2)-3=0,解得m=3或-1. 经过验证,m=3时两条直线重合,舍去. ∴m=-1. 直线l1:x+my+6=0与l2:(m-2)x+3y+2m=0分 别化为x-y+6=0, x y 2 0.
第二章 直线和圆的方程
2.3.4 两条平行直线间的距离
学习目标
两条平行直线间距离
1.掌握平面上两点间的距离公式.(数学抽象) 2.掌握点到直线的距离公式.(数学抽象) 3.会求两条平行直线间的距离.(数学运算) 4.会运用坐标法证明简单的平面几何问题.(数学建模)
新知探索
两条平行直线间距离
两条平行直线间的距离 1.概念:夹在两条平行直线间的公垂线段的长度就是两
解:(1)如图,显然有0<d≤|AB|.
y
| AB | (6 3)2 (2 1)2 3 10,
故所求的d的变化范围为 (0,3 10].
(2)当d取最大值时,两直线与AB垂直.
杨浦虹口五角场最好的初中数学补习班恒高一对一角的关系

『课堂练习』1.选择:
(1)两个三角形的两条边及其中一条边的对角对应相等,则下列四个命题中,真命题的个数是()个
①这两个三角形全等; ②相等的角为锐角时全等
③相等的角为钝角对全等; ④相等的角为直角时全等
A.0 B.1 C.2 D.3
(2)在下列定理中假命题是()
A.一个等腰三角形必能分成两个全等的直角三角形
B.一个直角三角形必能分成两个等腰三角形
C.两个全等的直角三角形必能拼成一个等腰三角形
D.两个等腰三角形必能拼成一个直角三角形
(3)如图,Rt△ABC中,∠B=90°,∠ACB=60°,延长BC到D,使CD=AC则AC:BD=()A.1:1 B.3:1 C.4:1 D.2:3
(4)如图,在Rt△ABC中,∠ACB=90°,CD、CE,分别是斜边AB上的高与中线,CF是∠ACB的平分线。
则∠1与∠2的关系是()
A.∠1<∠2 B.∠1=∠2; C.∠1>∠2 D.不能确定
(5)在直角三角形ABC中,若∠C=90°,D是BC边上的一点,且AD=2CD,则∠ADB的度数是()
A.30°B.60°C.120°D.150°
(6)(黄岛)下列条件中,能判定两个直角三角形全等的是()
A.一锐角对应相等B.两锐角对应相等
C.一条边对应相等D.两条直角边对应相等。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
『基础练习』
1、 填空题:
(1)在E O F ∠内部(包括顶点)到EOF ∠的两边距离相等的点的轨迹是__________________________________________________________________
(2)两直线AB 、CD 相交于O ,到这两条直线距离相等的点的轨迹是__________ ___________________________________________________________________
(3)边长BC = 4 cm ,面积S = 6 cm 2的三角形ABC 的顶点A 的轨迹是__________ ___________________________________________________________________
2、 是非题:
(1)因为线段AB 的垂直平分线上的点到线段AB 两个端点的距离相等,因此以AB 为底边的等腰三角形的顶角的顶点的轨迹是线段AB 的垂直平分线。
( )
(2)因为弧AB ⌒上的每一点到圆心O 的距离都等于半径R ,因此到点O 的距离等于半径R 的点的轨迹是AB ⌒。
( )
3、 在同一个平面内到定点O 的距离都等于2cm 的点的集合是怎样的一个图形?
4、作图并说明符合下列条件的点的轨迹(不要求证明)。
(1)经过已知点P 和Q 的圆的圆心的轨迹;
(2)到点A 的距离等于2cm 的点的轨迹;
(3)与已知直线AB 的距离为3cm 的点的轨迹。
. .
P Q A B。
