2017 AMC 8 考题及答案

美国数学竞赛AMC题目及答案

美国数学竞赛A M C题目及答案It was last revised on January 2, 20212.is the value offriends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $ to cover her portion of the total bill. What was the total billis in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many poundsnumber in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top rowand his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the trainfair coin is tossed 3 times. What is the probability of at least two consecutive headsIncredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometeris the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 59411. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40%discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save13. When Clara totaled her scores, she inadvertently reversed the units digit and thetens digit of one score. By which of the following might her incorrect sum have differed from the correct one14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the differenceis a multiple of 9, the only answer choice that is a multiple of 9 is .15. If , , and , what is the product of , , and16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain-- 16:22, 27 November 2013 (EST) Courtesy of19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'Ididn't get the highest score.' What is the ranking of the three girls from highest to lowest20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle21. Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take22. Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether23. Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length . What is the radius of the semicircle on24. Squares , , and are equal in area. Points and are the midpoints of sides and , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares25. A ball with diameter 4 inches starts at point A to roll along the track shown. Thetrack is comprised of 3 semicircular arcs whose radii are inches,inches, and inches, respectively. The ball always remains in contactwith the track and does not slip. What is the distance the center of the ball travels over the course from A to B1.50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound offish is dollars.that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be.median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer.1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number.This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groupsof cars that Trey most likely counts. Since , the closest answer choiceis ., there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11. We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore,.On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked atper day. Set up the equation, .To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have .Then, find the amount of money using the discount: . Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the differenceis a multiple of 9, the only answer choice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is.Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7thgraders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are, so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there are cubes. Hence, the answer is.Solution 2We can just calculate the volume of the prism that was cut out of the originalbox. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number ofblocks contained in the fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class. Therefore, Hannah did better thanBridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and thenumber of ways to get from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22. There are vertical columns with a length of toothpicks, and there arehorizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, . a grid of toothpicks. Thus, our answer is.23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagorean theorem says that the other side has length 15, so theradius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equalto the biggest one, so the area of the largest is , and the middle one is, so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits the extension of . Call this point . The area of triangle then isThe area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to the combined area of the three squares is.Solution 2Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined withyields .The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Solution 3Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore . Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and itgains inches on B. So, the departure from the length of the track means that the answer is.Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choiceless than is . This solution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.。

AMC考题和答案解析

A M C考题和答案解析Document serial number【NL89WT-NY98YT-NC8CB-NNUUT-NUT108】2017 AMC 8 考题及答案Problem 1Which of the following values is largestProblem 2Alicia, Brenda, and Colby were the candidates in a recent election for student president. The pie chart below shows how the votes were distributed among the three candidates. If Brenda received 36 votes, then how many votes were cast all togetherProblem 3What is the value of the expressionProblem 4When is multiplied by 7,928,564 the product is closest to which of the followingProblem 5What is the value of the expressionProblem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of the triangleProblem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor ofProblem 8Malcolm wants to visit Isabella after school today and knows the street where she lives but doesn't know her house number. She tellshim, "My house number has two digits, and exactly three of the following four statements about it are true."(1) It is prime.(2) It is even.(3) It is divisible by 7.(4) One of its digits is 9.This information allows Malcolm to determine Isabella's house number. What is its units digitProblem 9All of Marcy's marbles are blue, red, green, or yellow. One third of her marbles are blue, one fourth of them are red, and six of them are green. What is the smallest number of yellow marbles that Marcy could haveProblem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is the probability that 4 is the largest value selectedProblem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floorProblem 12The smallest positive integer greater than 1 that leaves a remainderof 1 when divided by 4, 5, and 6 lies between which of the following pairs of numbersProblem 13Peter, Emma, and Kyler played chess with each other. Peter won 4 games and lost 2 games. Emma won 3 games and lost 3 games. If Kyler lost 3 games, how many games did he winProblem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Last night they each solved half of the problems in their homework assignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answers to of the problems she solved alone. What was Zoe's overall percentage of correct answersProblem 15In the arrangement of letters and numerals below, by how manydifferent paths can one spell AMC8 Beginning at the A in the middle,a path allows only moves from one letter to an adjacent (above, below,left, or right, but not diagonal) letter. One example of such a path is traced in the picture.Problem 16In the figure below, choose point on so that and have equal perimeters. What is the area ofProblem 17Starting with some gold coins and some empty treasure chests, I tried to put 9 gold coins in each treasure chest, but that left 2 treasurechests empty. So instead I put 6 gold coins in each treasure chest, but then I had 3 gold coins left over. How many gold coins did I haveProblem 18In the non-convex quadrilateral shown below, is a right angle, , , , and .What is the area of quadrilateralProblem 19For any positive integer , the notation denotes the product of the integers through . What is the largest integer for whichis a factor of the sumProblem 20An integer between and , inclusive, is chosen at random. What is the probability that it is an odd integer whose digits are all distinctProblem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) forProblem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is the radius of the semicircleProblem 23Each day for four days, Linda traveled for one hour at a speed that resulted in her traveling one mile in an integer number of minutes. Each day after the first, her speed decreased so that the number of minutes to travel one mile increased by 5 minutes over the preceding day. Each of the four days, her distance traveled was also an integer number of miles. What was the total number of miles for the fourtripsProblem 24Mrs. Sanders has three grandchildren, who call her regularly. One calls her every three days, one calls her every four days, and one calls her every five days. All three called her on December 31, 2016. On how many days during the next year did she not receive a phonecall from any of her grandchildrenProblem 25In the figure shown, and are line segments each of length 2, and . Arcs and are each one-sixth of a circle with radius 2. What is the area of the region shown2017 AMC 8 Answer Key1.A2.E3.C4.D5.B6.D7.A8.D9.D10.C11.C12.D13.B14.C15.D16.D17.C18.B19.D20.B21.A22.D23.C24.D25.B。

2017 AMC 8 考题及答案Problem 1Which of the following values is largest?Problem 2Alicia, Brenda, and Colby were the candidates in a recent election for student president. The pie chart below shows how the votes were distributed among the three candidates. If Brenda received 36 votes, then how many votes were cast all together?Problem 3What is the value of the expression ?Problem 4When 0.000315 is multiplied by 7,928,564 the product is closest to which of the following?Problem 5What is the value of the expression ?Problem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of the triangle?Problem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor of ?Problem 8Malcolm wants to visit Isabella after school today and knows the street where she lives but doesn't know her house number. She tells him, "My house number has two digits, and exactly three of the following four statements about it are true."(1) It is prime.(2) It is even.(3) It is divisible by 7.(4) One of its digits is 9.This information allows Malcolm to determine Isabella's house number. What is its units digit?Problem 9All of Marcy's marbles are blue, red, green, or yellow. One third of her marbles are blue, one fourth of them are red, and six of them are green. What is the smallest number of yellow marbles that Marcy could have?Problem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is the probability that 4 is the largest value selected?Problem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floor?Problem 12The smallest positive integer greater than 1 that leaves a remainder of 1 when divided by 4, 5, and 6 lies between which of the following pairs of numbers?Problem 13Peter, Emma, and Kyler played chess with each other. Peter won 4 games and lost 2 games. Emma won 3 games and lost 3 games. If Kyler lost 3 games, how many games did he win?Problem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Last night they each solved half of the problems in their homework assignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answers to of the problems she solved alone. What was Zoe's overall percentage of correct answers?Problem 15In the arrangement of letters and numerals below, by how many different paths can one spell AMC8? Beginning at the A in the middle, a path allows only moves from one letter to an adjacent (above, below, left, or right, but not diagonal) letter. One example of such a path is traced in the picture.Problem 16In the figure below, choose point on so that and have equal perimeters. What is the area of ?Problem 17Starting with some gold coins and some empty treasure chests, I tried to put 9 gold coins in each treasure chest, but that left 2 treasure chests empty. So instead I put 6 gold coins in each treasure chest, but then I had 3 gold coins left over. How many gold coins did I have?Problem 18In the non-convex quadrilateral shown below, is a right angle, , , , and .What is the area of quadrilateral ?For any positive integer , the notation denotes the product of the integers through . What is the largest integer for which is a factor of the sum ?Problem 20An integer between and , inclusive, is chosen at random. What is the probability that it is an odd integer whose digits are all distinct?Problem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) for ?Problem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is the radius of the semicircle?Each day for four days, Linda traveled for one hour at a speed that resulted in her traveling one mile in an integer number of minutes. Each day after the first, her speed decreased so that the number of minutes to travel one mile increased by 5 minutes over the preceding day. Each of the four days, her distance traveled was also an integer number of miles. What was the total number of miles for the four trips?Problem 24Mrs. Sanders has three grandchildren, who call her regularly. One calls her every three days, one calls her every four days, and one calls her every five days. All three called her on December 31, 2016. On how many days during the next year did she not receive a phone call from any of her grandchildren?Problem 25In the figure shown, and are line segments each of length 2, and . Arcs and are each one-sixth of a circle with radius 2. What is the area of the region shown?2017 AMC 8 Answer Key1. A2. E3. C4. D5. B6. D7. A8. D9. D10.C11.C12.D13.14.B15.16.C17.18.D19.20.D21.22.C23.24.B25.D26.B27.28.A29.30.D31.32.C33.D34.35.B。

美国数学竞赛AMC题目及答案

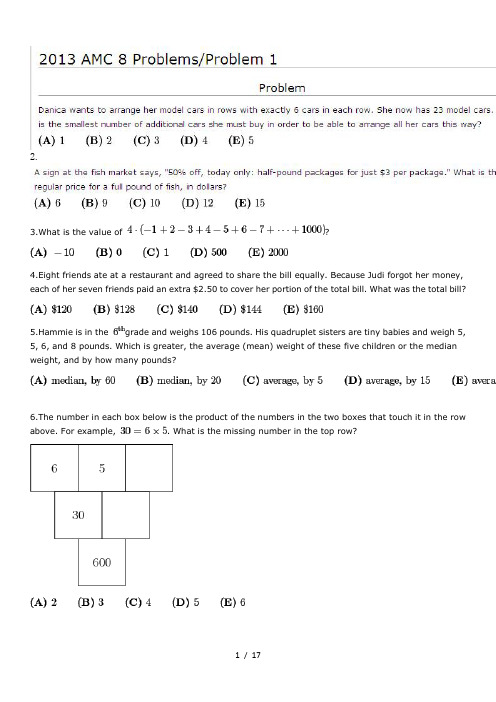
2.3.What is the value of ?4.Eight friends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $2.50 to cover her portion of the total bill. What was the total bill?5.Hammie is in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many pounds?6.The number in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top row?7.Trey and his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the train?8.A fair coin is tossed 3 times. What is the probability of at least two consecutive heads?9.The Incredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometer?10.What is the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 594?11. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less?12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save?13. When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one?14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .15. If , , and , what is the product of , , and ?16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project?17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers?18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain?--Arpanliku 16:22, 27 November 2013 (EST) Courtesy of Lord.of.AMC19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest?20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle?21. Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take?22. Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether?23. Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length .What is the radius of the semicircle on ?24. Squares , , and are equal in area. Points and are the midpoints of sidesand , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares?25. A ball with diameter 4 inches starts at point A to roll along the track shown. The track is comprised of 3 semicircular arcs whose radii are inches, inches, and inches, respectively. The ball always remains in contact with the track and does not slip. What is the distance the center of theball travels over the course from A to B?1.2.The 50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound of fish is dollars.3.Notice that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .4.Each of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be .5.The median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer.6.Solution 1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number. This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:7.If Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groups of cars that Trey most likely counts. Since , the closest answer choice is .8.First, there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .9.This is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11. We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore, .On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked at per day. Set up the equation, .To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have.Then, find the amount of money using the discount: .Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is .Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7th graders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are, so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there arecubes. Hence, the answer is .Solution 2We can just calculate the volume of the prism that was cut out of the original box. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number of blocks contained inthe fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class.Therefore, Hannah did better than Bridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and the number of ways toget from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22. There are vertical columns with a length of toothpicks, and there are horizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, i.e. a grid of toothpicks.Thus, our answer is .23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagoreantheorem says that the other side has length 15, so the radius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equal to the biggest one, so the area of thelargest is , and the middle one is , so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits theextension of . Call this point . The area of triangle then is The area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to thecombined area of the three squares is .Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined with yields.The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore .Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and it gains inches on B.So, the departure from the length of the track means that the answer is . Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choice less than is . Thissolution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.古希腊哲学大师亚里士多德说：人有两种，一种即“吃饭是为了活着”,一种是“活着是为了吃饭”.一个人之所以伟大，首先是因为他有超于常人的心。

美国数学竞赛AMC8 -- 2010年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 -- 2010年真题解析(英文解析+中文解析)Problem 1Answer: CSolution:Given that these are the only math teachers at Euclid Middle School and we are told how many from each class are taking the AMC 8, we simply add the three numbers to find the total.11+8+9=28.中文解析:参加竞赛的学生总人数是：11+8+9=28. 答案是C。

Problem 2Answer: DSolution:Substitute a=5, b=10 into the expression for a@b to get: 5@10=(5*10)/(5+10)=50/15=10/3.中文解析：（5*10）/（5+10）=50/15=10/3. 答案是D。

Problem 3Answer: CSolution:The highest price was in Month 1, which was $17. The lowest price was in Month 3, which was $10. 17 is 17/10 =170% of 10, and is 170-100=70% more than 10. Therefore, the answer is 70. 中文解析：最高价是1月，17美元。

最低价格是3月10美元。

最高价比最低价多：（17-10）/10=70%。

答案是C。

Problem 4Answer: CSolution:Putting the numbers in numerical order we get the list 0,0,1,2,3,3,3,4 The mode is 3, The median is (2+3)/2=2.5. The average is 2. is The sum of all three is 3+2.5+2=7.5.中文解析：这组数按照从小到大的顺序排列是：0，0，1，2，3，3，3，4. 中位数Median是2.5；mode 是3，mean是16/8=2. 因此mean,median,mode的和是: 2.5+3+2=7.5. 答案是C。

美国数学竞赛AMC题目及答案

2.3.Whatisthevalueof4.5.Hammieisinthegradeandweighs106pounds.Hisquadrupletsistersaretinybabiesandweigh5,5,6,and8pounds.W hichisgreater,theaverage(mean)weightofthesefivechildrenorthemedianweight,andbyhowman ypounds6.Thenumberineachboxbelowistheproductofthenumbersinthetwoboxesthattouchitintherowab ove.Forexample,.Whatisthemissingnumberinthetoprow7.8.Afaircoinistossed3times.Whatistheprobabilityofatleasttwoconsecutiveheads9.TheIncredibleHulkcandoublethedistancehejumpswitheachsucceedingjump.Ifhisfirstjumpis1 meter,thesecondjumpis2meters,thethirdjumpis4meters,andsoon,thenonwhichjumpwillhefirst beabletojumpmorethan1kilometer10.Whatistheratiooftheleastcommonmultipleof180and594tothegreatestcommonfactorof180a nd59411.12.Atthe2013WinnebagoCountyFairavendorisofferinga"fairspecial"onsandals.Ifyoubuyonepai rofsandalsattheregularpriceof$50,yougetasecondpairata40%discount,andathirdpairathalfthe regularprice.Javiertookadvantageofthe"fairspecial"tobuythreepairsofsandals.Whatpercentag eofthe$150regularpricedidhesave13.WhenClaratotaledherscores,sheinadvertentlyreversedtheunitsdigitandthetensdigitofones core.Bywhichofthefollowingmightherincorrectsumhavedifferedfromthecorrectone14.Letthetwodigitsbe and.Thecorrectscorewas.Claramisinterpreteditas.Thedifferencebetweenthetwoiswhichfactorsinto.Therefore,sincethedifferenceisamultipleof9,theonlyanswerchoicethatisamultipleof9 is.15.If,,and,whatistheproductof,,and16.AnumberofstudentsfromFibonacciMiddleSchoolaretakingpartinacommunityserviceproject.Theratioof-gradersto-gradersis,andthetheratioof-gradersto-gradersis .Whatisthesmallestnumberofstudentsthatcouldbeparticipatingintheproject17.Thesumofsixconsecutivepositiveintegersis2013.Whatisthelargestofthesesixintegers18.--Arpanliku16:22,27November2013(EST)Courtesyof19.Bridget,Cassie,andHannaharediscussingtheresultsoftheirlastmathtest.HannahshowsBridg etandCassiehertest,butBridgetandCassiedon'tshowtheirstoanyone.Cassiesays,'Ididn'tgetthel owestscoreinourclass,'andBridgetadds,'Ididn'tgetthehighestscore.'Whatistherankingofthethr eegirlsfromhighesttolowest20.Arectangleisinscribedinasemicirclewithlongersideonthediameter.Whatistheareaofthesemicircle21.22.Toothpicksareusedtomakeagridthatis60toothpickslongand32toothpickswide.Howmanytoo thpicksareusedaltogether23.Angle of isarightangle.Thesidesofarethediametersofsemicirclesasshown.Theareaofthesemicircleon equals,andthearcofthesemicircleon haslength.Whatistheradiusofthesemicircleon24.Squares,,and areequalinarea.Points andarethemidpointsofsides and,respectively.Whatistheratiooftheareaoftheshadedpentagontothesumoftheareasofthethreesquares25.Aballwithdiameter4inchesstartsatpointAtorollalongthetrackshown.Thetrackiscomprisedof3semicirculararcswhoseradiiare inches,inches,and1.2.The50%offpriceofhalfapoundoffishis$3,sothe100%,ortheregularprice,ofahalfpoundoffishis$6.Consequently,ifhalfapoundoffishcosts$6,thenawholepoundoffishis dollars.3.Noticethatwecanpairupeverytwonumberstomakeasumof1:Therefore,theansweris.4.Eachofhersevenfriendspaid tocoverJudi'sportion.Therefore,Judi'sportionmustbe.SinceJudiwassupposedtopay ofthetotalbill,thetotalbillmustbe.5.Themedianhereisobviouslylessthanthemean,sooption(A)and(B)areout. Liningupthenumbers(5,5,6,8,106),weseethatthemedianweightis6pounds.Theaverageweightofthefivekidsis.Therefore,theaverageweightisbigger,by pounds,makingtheanswer.6.Solution1:WorkingBackwardsLetthevalueintheemptyboxinthemiddlerowbe,andthevalueintheemptyboxinthetoprowbe. istheanswerwe'relookingfor.Weseethat,making.Itfollowsthat,so.Solution2:JumpingBacktotheStartAnotherwaytodothisproblemistorealizewhatmakesupthebottommostnumber.Thismethoddoes n'tworkquiteaswellforthisproblem,butinalargertree,itmightbefaster.(Inthiscase,Solution1wou ldbefastersincethere'sonlytwomissingnumbers.)Again,letthevalueintheemptyboxinthemiddlerowbe,andthevalueintheemptyboxinthetoprowbe.istheanswerwe'relookingfor. Wecanwritesomeequations: Nowwecansubstituteintothefirstequationusingthetwoothers:7.IfTreysaw,thenhesaw.2minutesand45secondscanalsobeexpressedas seconds.Trey'srateofseeingcars,,canbemultipliedbyonthetopandbottom(andpreservethesamerate):.Itfollowsthatthemostlikelynumberofcarsis. Solution2minutesand secondsisequalto.SinceTreyprobablycountsaround carsevery seconds,thereare groupsofcarsthatTreymostlikelycounts.Since,theclosestanswerchoiceis.8.First,thereare waystoflipthecoins,inorder. ThewaystogettwoconsecutiveheadsareHHT andTHH. ThewaytogetthreeconsecutiveheadsisHHH.Therefore,theprobabilityofflippingatleasttwoconsecutiveheadsis.9.Thisisageometricsequenceinwhichthecommonratiois2.Tofindthejumpthatwouldbeovera1000meters,wenotethat.However,becausethefirsttermis andnot,thesolutiontotheproblemis10.TofindeithertheLCMortheGCFoftwonumbers,alwaysprimefactorizefirst. Theprimefactorizationof.Theprimefactorizationof.Then,findthegreatestpowerofallthenumbersthereare;ifonenumberisonebutnottheother,useit(t hisis).Multiplyallofthesetoget5940.FortheGCFof180and594,usetheleastpowerofallofthenumbersthatareinbothfactorizationsandmultiply.=18.Thustheanswer==. Westartoffwithasimilarapproachastheoriginalsolution.Fromtheprimefactorizations,theGCFis .Itisawellknownfactthat.Sowehave,.Dividingby yields.Therefore,.11.Weusethatfactthat.Letd=distance,r=rateorspeed,andt=time.Inthiscase,letrepresentthetime.OnMonday,hewasatarateof.So,.ForWednesday,hewalkedatarateof.Therefore,.OnFriday,hewalkedatarateof.So,.Addingupthehoursyields++=.WenowfindtheamountoftimeGrandfatherwouldhavetakenifhewalkedatperday.Setuptheequation,.Tofindtheamountoftimesaved,subtractthetwoamounts:-=.Toconvertthistominutes,wemultiplyby.Thus,thesolutiontothisproblemis12.First,findtheamountofmoneyonewillpayforthreesandalswithoutthediscount.Wehave.Then,findtheamountofmoneyusingthediscount:.Findingthepercentageyields.Tofindthepercentsaved,wehave13.Letthetwodigitsbe and.Thecorrectscorewas.Claramisinterpreteditas.Thedifferencebetweenthetwoiswhichfactorsinto.Therefore,sincethedifferenceisamultipleof9,theonlyanswerchoicethatisamultipleof9is.14.Theprobabilitythatbothshowagreenbeanis.Theprobabilitythatbothshowaredbeanis.Thereforetheprobabilityis15.Therefore,.Therefore,.Tomostpeople,itwouldnotbeimmediatelyevidentthat,sowecanmultiply6'suntilwegetthedesirednumber:,so.Thereforetheansweris.16.Solution1:AlgebraWemultiplythefirstratioby8onbothsides,andthesecondratioby5togetthesamenumberfor8thgra ders,inorderthatwecanputthetworatiostogether:Therefore,theratioof8thgradersto7thgradersto6thgradersis.Sincetheratioisinlowestterms,thesmallestnumberofstudentsparticipatingintheprojectis.Solution2:FakesolvingThenumberof8thgradershastobeamultipleof8and5,soassumeitis40(thesmallestpossibility).Thenthereare6thgradersand7thgraders.Thenumbersofstudentsis17.Solution1Themeanofthesenumbersis.Thereforethenumbersare,sotheanswerisSolution2Letthe numberbe.Thenourdesirednumberis.Ourintegersare,sowehavethat.Solution3Letthefirsttermbe.Ourintegersare.Wehave,18.Solution1Therearecubesonthebaseofthebox.Then,foreachofthe4layersabovethebottom(assinceeachcubeis1foot by1footby1footandtheboxis5feettall,thereare4feetleft),therearecubes.Hence,theansweris.Solution2Wecanjustcalculatethevolumeoftheprismthatwascutoutoftheoriginalbox.Eachinteriorsideofthefortwillbe feetshorterthaneachsideoftheoutside.Sincethefloorisfoot,theheightwillbe feet.Sothevolumeoftheinteriorboxis.Thevolumeoftheoriginalboxis.Therefore,thenumberofblockscontainedinthefortis.19.IfHannahdidbetterthanCassie,therewouldbenowayshecouldknowforsurethatshedidn'tgett helowestscoreintheclass.Therefore,HannahdidworsethanCassie.Similarly,ifHannahdidworseth anBridget,thereisnowayBridgetcouldhaveknownthatshedidn'tgetthehighestintheclass.Therefore,HannahdidbetterthanBridget,soourorderis.20.Asemicirclehassymmetry,sothecenterisexactlyatthemidpointofthe2sideontherectangle,makingtheradius,bythePythagoreanTheorem,.Theareais.21.ThenumberofwaystogetfromSamantha'shousetoCityParkis,andthenumberofwaystogetfromCityParktoschoolis.Sincethere'sonewaytogothroughCityPark(justwalkingstraightthrough),thenumberofdifferentwaystogofromSamantha'shousetoCityParktoschool.22.Thereare verticalcolumnswithalengthof toothpicks,andthereare horizontalrowswithalengthof toothpicks.Aneffectivewaytoverifythisistotryasmallcase,i.e.agridoftoothpicks.Thus,ouransweris.23.Solution1.Solution2WegoasinSolution1,findingthediameterofthecircleonACandAB.Then,anextendedversionofthet heoremsaysthatthesumofthesemicirclesontheleftisequaltothebiggestone,sotheareaofthelargestis,andthemiddleoneis,sotheradiusis.24.Firstlet(where isthesidelengthofthesquares)forsimplicity.Wecanextenduntilithitstheextensionof.Callthispoint.Theareaoftriangle thenis Theareaofrectangle is.Thus,ourdesiredareais.Now,theratiooftheshadedareatothecombinedareaofthethreesquaresis.Solution2Letthesidelengthofeachsquarebe.Lettheintersectionof and be.Since,.Since andareverticalangles,theyarecongruent.Wealsohave bydefinition.Sowehave by congruence.Therefore,.Since and aremidpointsofsides,.Thiscombinedwith yields.Theareaoftrapezoid is.Theareaoftriangle is.Sotheareaofthepentagon is.Theareaofthe squaresis.Therefore,.Solution3Lettheintersectionof and be.Nowwehave and.Becausebothtriangleshasasideoncongruentsquarestherefore.Because and areverticalangles.Alsoboth and arerightanglesso.ThereforebyAAS(Angle,Angle,Side).Thentranslating/rotatingtheshaded intothepositionofSotheshadedareanowcompletelycoversthesquareSettheareaofasquareasTherefore,.25.Solution1Theradiusoftheballis2inches.Ifyouthinkabouttheballrollingordrawapathfortheball(seefigurebelow),youseethatinAandCitloses inches,anditgains inchesonB.So,thedeparturefromthelengthofthetrackmeansthattheansweris.Solution2Thetotallengthofallofthearcsis.Sincewewantthepathfromthecenter,theactualdistancewillbeshorter.Therefore,theonlyanswerchoicelessthan is.Thissolutionmaybeinvalidbecausetheactualdistancecanbelongerifthepaththecent ertravelsisontheoutsideofthecurve,asitisinthemiddlebump.。

美国数学竞赛AMC题目及答案

2.is the value offriends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $ to cover her portion of the total bill. What was the total billis in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many poundsnumber in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top rowand his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the trainfair coin is tossed 3 times. What is the probability of at least two consecutive headsIncredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometeris the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 59411. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save13.When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answer choice that is a multiple of 9 is .15. If , , and , what is the product of , , and16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain--Arpanliku 16:22, 27 November 2013 (EST) Courtesy of19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle21.Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take22.Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether23.Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length . What isthe radius of the semicircle on24. Squares , , and are equal in area. Points and are the midpoints of sidesand , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares25. A ball with diameter 4 inches starts at point A to roll along the track shown. The track is comprised of 3 semicircular arcs whose radii are inches, inches, and inches, respectively. The ball always remains in contact with the track and does not slip. What is the distance the center of theball travels over the course from A to B1.50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound of fish is dollars.that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be .median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer .1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number. This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groups of cars that Trey most likely counts. Since , the closest answer choice is ., there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11.We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore, .On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked at per day. Set up the equation,.To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have.Then, find the amount of money using the discount: .Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answer choice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is .Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7th graders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are , so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there are cubes. Hence, the answer is .Solution 2We can just calculate the volume of the prism that was cut out of the original box. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number of blocks contained in the fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class. Therefore, Hannah did better than Bridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and the number of ways to get from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22.There are vertical columns with a length of toothpicks, and there are horizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, . a grid of toothpicks. Thus, our answer is .23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagorean theorem says that the other side has length 15, so the radius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equal to the biggest one, so the area of the largestis , and the middle one is , so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits the extension of . Call this point . The area of triangle then is The area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to the combinedarea of the three squares is .Solution 2Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined with yields.The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Solution 3Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore .Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and it gains inches on B.So, the departure from the length of the track means that the answer is . Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choice less than is . This solution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.。

AMC8(美国数学竞赛)历年真题、答案及中英文解析

AMC8（美国数学竞赛）历年真题、答案及中英文解析艾蕾特教育的AMC8 美国数学竞赛考试历年真题、答案及中英文解析：AMC8-2020年：真题 --- 答案---解析（英文解析+中文解析）AMC8 - 2019年：真题----答案----解析（英文解析+中文解析）AMC8 - 2018年：真题----答案----解析（英文解析+中文解析）AMC8 - 2017年：真题----答案----解析（英文解析+中文解析）AMC8 - 2016年：真题----答案----解析（英文解析+中文解析）AMC8 - 2015年：真题----答案----解析（英文解析+中文解析）AMC8 - 2014年：真题----答案----解析（英文解析+中文解析）AMC8 - 2013年：真题----答案----解析（英文解析+中文解析）AMC8 - 2012年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 2010年：真题----答案----解析（英文解析+中文解析）AMC8 - 2009年：真题----答案----解析（英文解析+中文解析）AMC8 - 2008年：真题----答案----解析（英文解析+中文解析）AMC8 - 2007年：真题----答案----解析（英文解析+中文解析）AMC8 - 2006年：真题----答案----解析（英文解析+中文解析）AMC8 - 2005年：真题----答案----解析（英文解析+中文解析）AMC8 - 2004年：真题----答案----解析（英文解析+中文解析）AMC8 - 2003年：真题----答案----解析（英文解析+中文解析）AMC8 - 2002年：真题----答案----解析（英文解析+中文解析）AMC8 - 2001年：真题----答案----解析（英文解析+中文解析）AMC8 - 2000年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1998年：真题----答案----解析（英文解析+中文解析）AMC8 - 1997年：真题----答案----解析（英文解析+中文解析）AMC8 - 1996年：真题----答案----解析（英文解析+中文解析）AMC8 - 1995年：真题----答案----解析（英文解析+中文解析）AMC8 - 1994年：真题----答案----解析（英文解析+中文解析）AMC8 - 1993年：真题----答案----解析（英文解析+中文解析）AMC8 - 1992年：真题----答案----解析（英文解析+中文解析）AMC8 - 1991年：真题----答案----解析（英文解析+中文解析）AMC8 - 1990年：真题----答案----解析（英文解析+中文解析）AMC8 - 1989年：真题----答案----解析（英文解析+中文解析）AMC8 - 1988年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1986年：真题----答案----解析（英文解析+中文解析）AMC8 - 1985年：真题----答案----解析（英文解析+中文解析）◆AMC介绍◆AMC（American Mathematics Competitions) 由美国数学协会（MAA）组织的数学竞赛，分为 AMC8 、 AMC10、 AMC12 。

2017-2020AMC真题和答案

2020 AMC 12A Problems Problem 1Carlos took of a whole pie. Maria took one third of the remainder. What portion of the whole pie was left?Problem 2The acronym AMC is shown in the rectangular grid below with grid linesspaced unit apart. In units, what is the sum of the lengths of the line segments that form the acronym AMCProblem 3A driver travels for hours at miles per hour, during which her cargets miles per gallon of gasoline. She is paid per mile, and her only expense is gasoline at per gallon. What is her net rate of pay, in dollars per hour, after this expense?Problem 4How many -digit positive integers (that is, integers between and , inclusive) having only even digits are divisible byProblem 5The integers from to inclusive, can be arranged to form a -by-square in which the sum of the numbers in each row, the sum of the numbers in each column, and the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum?Problem 6In the plane figure shown below, of the unit squares have been shaded. What is the least number of additional unit squares that must be shaded so that the resulting figure has two lines of symmetryProblem 7Seven cubes, whose volumes are , , , , , , and cubic units, are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top. Except for the bottom cube, the bottom face of each cube lies completely on top of the cube below it. What is the total surface area of the tower (including the bottom) in square units?Problem 8What is the median of the following list of numbersProblem 9How many solutions does the equation have on the intervalProblem 10There is a unique positive integer suchthat What is the sum of the digitsofProblem 11A frog sitting at the point begins a sequence of jumps, where each jump is parallel to one of the coordinate axes and has length , and the direction of each jump (up, down, right, or left) is chosen independently at random. The sequence ends when the frog reaches a side of the square withvertices and . What is the probability that thesequence of jumps ends on a vertical side of the squareProblem 12Line in the coordinate plane has the equation . Thisline is rotated counterclockwise about the point to obtain line . What is the -coordinate of the -intercept of lineProblem 13There are integers , , and , each greater than 1, suchthat for all . What is ?Problem 14Regular octagon has area . Let be the area ofquadrilateral . What isProblem 15In the complex plane, let be the set of solutions to andlet be the set of solutions to . What is the greatest distance between a point of and a point ofProblem 16A point is chosen at random within the square in the coordinate plane whose vertices are and . Theprobability that the point is within units of a lattice point is . (A point is a lattice point if and are both integers.) What is to the nearest tenthProblem 17The vertices of a quadrilateral lie on the graph of , and the -coordinates of these vertices are consecutive positive integers. The area of thequadrilateral is . What is the -coordinate of the leftmost vertex?Problem 18Quadrilateral satisfies, and . Diagonals and intersect at point , and . What is the area of quadrilateral ?Problem 19There exists a unique strictly increasing sequence of nonnegativeintegers suchthat What isProblem 20Let be the triangle in the coordinate plane with vertices , ,and . Consider the following five isometries (rigid transformations) of the plane: rotations of , , and counterclockwise around the origin, reflection across the -axis, and reflection across the -axis. How many ofthe sequences of three of these transformations (not necessarily distinct) will return to its original position? (For example, a rotation, followed by a reflection across the -axis, followed by a reflection across the -axis will return to its original position, but a rotation, followed by a reflection across the -axis, followed by another reflection across the -axis will not return to its original position.)Problem 21How many positive integers are there such that is a multiple of , and the least common multiple of and equals times the greatest common divisor of andProblem 22Let and be the sequences of real numbers suchthat for all integers , where . What isProblem 23Jason rolls three fair standard six-sided dice. Then he looks at the rolls and chooses a subset of the dice (possibly empty, possibly all three dice) to reroll. After rerolling, he wins if and only if the sum of the numbers face up on the three dice is exactly . Jason always plays to optimize his chances of winning. What is the probability that he chooses to reroll exactly two of the dice?Problem 24Suppose that is an equilateral triangle of side length , with the property that there is a unique point inside the triangle suchthat , , and . What isProblem 25The number , where and are relatively prime positive integers, has the property that the sum of all realnumbers satisfying is , where denotes thegreatest integer less than or equal to and denotes the fractional part of . What is2020 AMC 12A Answer Key1. C2. C3. E4. B5. C6. D7. B8. C9. E10.E11.B12.B13.B14.B15.D16.B17.D18.D19.C20.A21.D22.B23.A24.B25.C2020 AMC 12B Problems Problem 1What is the value in simplest form of the followingexpression?Problem 2What is the value of the followingexpression?Problem 3The ratio of to is , the ratio of to is , and the ratioof to is . What is the ratio of to ?Problem 4The acute angles of a right triangle are and , where andboth and are prime numbers. What is the least possible value of ?Problem 5Teams and are playing in a basketball league where each game results in a win for one team and a loss for the other team. Team has won of itsgames and team has won of its games. Also, team has won moregames and lost more games than team How many games hasteam played?Problem 6For all integers the value of is always whichof the following?Problem 7Two nonhorizontal, non vertical lines in the -coordinate plane intersect to form a angle. One line has slope equal to times the slope of the other line. What is the greatest possible value of the product of the slopes of the two lines?Problem 8How many ordered pairs of integers satisfy theequationProblem 9A three-quarter sector of a circle of radius inches together with its interior can be rolled up to form the lateral surface area of a right circular cone by tapingtogether along the two radii shown. What is the volume of the cone in cubicinches?Problem 10In unit square the inscribedcircle intersects at and intersects at a point different from What isProblem 11As shown in the figure below, six semicircles lie in the interior of a regular hexagon with side length so that the diameters of the semicircles coincide with the sides of the hexagon. What is the area of the shaded region—inside the hexagon but outside all of the semicircles?Problem 12Let be a diameter in a circle of radius Let be a chord in the circle that intersects at a point suchthat and What isProblem 13Which of the following is the value ofProblem 14Bela and Jenn play the following game on the closed interval of the real number line, where is a fixed integer greater than . They take turns playing, with Bela going first. At his first turn, Bela chooses any real number in theinterval . Thereafter, the player whose turn it is chooses a real numberthat is more than one unit away from all numbers previously chosen by either player. A player unable to choose such a number loses. Using optimal strategy, which player will win the game?Problem 15There are 10 people standing equally spaced around a circle. Each person knows exactly 3 of the other 9 people: the 2 people standing next to her or him, as well as the person directly across the circle. How many ways are there for the 10 people to split up into 5 pairs so that the members of each pair know each other?Problem 16An urn contains one red ball and one blue ball. A box of extra red and blue balls lie nearby. George performs the following operation four times: he draws a ball from the urn at random and then takes a ball of the same color from the box and returns those two matching balls to the urn. After the four iterations the urn contains six balls. What is the probability that the urn contains three balls of each color?How many polynomials of theform , where , , ,and are real numbers, have the property that whenever is a root, sois ? (Note that )Problem 18In square , points and lie on and , respectively, so that Points and lie on and , respectively, and points and lie on so that and . See the figure below. Triangle , quadrilateral ,quadrilateral , and pentagon each has area Whatis ?Square in the coordinate plane has vertices at thepoints and Consider the following four transformations: a rotation of counterclockwise around the origin; a rotation of clockwise around the origin; a reflection across the -axis; and a reflection across the -axis.Each of these transformations maps the squares onto itself, but the positions of the labeled vertices will change. For example, applying and then wouldsend the vertex at to and would send thevertex at to itself. How many sequences of transformations chosen from will send all of the labeled vertices back to theiroriginal positions? (For example, is one sequenceof transformations that will send the vertices back to their original positions.)Problem 20Two different cubes of the same size are to be painted, with the color of each face being chosen independently and at random to be either black or white. What is the probability that after they are painted, the cubes can be rotated to be identical in appearance?Problem 21How many positive integers satisfy(Recallthat is the greatest integer not exceeding .)Problem 22What is the maximum value of for real values ofProblem 23How many integers are there such that whenever are complex numbers such thatthen the numbers are equally spaced on the unit circle in the complex plane?Problem 24Let denote the number of ways of writing the positive integer as a product where , the are integers strictly greater than , and the order in which the factors are listed matters (that is, two representations that differ only in the order of the factors are counted as distinct).For example, the number can be written as , , and ,so . What is ?Problem 25For each real number with , let numbers and be chosen independently at random from the intervals and , respectively, and let be the probability thatWhat is the maximum value of2020 AMC 12B Answer Key1. C2. A3. E4. D5. C6. D7. C8. D9. C10. B11. D12. E13. D14. A15. C16. B17. C18. B19. C20. D21. C22. C23. B24. A25. BThe area of a pizza with radius is percent larger than the area of a pizza with radius inches. What is the integer closest to ?Solution Supposeis of . What percent of is ?Solution A box containsred balls,green balls, yellow balls,blue balls, white balls, and black balls. What is the minimum numberof balls that must be drawn from the box without replacement to guarantee that at least balls of a single color will be drawn?SolutionWhat is the greatest number of consecutive integers whose sum is ?SolutionTwo lines with slopes andintersect at . What is the area of the triangle enclosed by these two lines and the line?SolutionThe ﬁgure below shows line with a regular, inﬁnite, recurring pattern of squares and line segments.How many of the following four kinds of rigid motion transformations of the plane in which this ﬁgure is drawn, other than the identity transformation, will transform this ﬁgure into itself?some rotation around a point of linesome translation in the direction parallel to linethe reﬂection across linesome reﬂection across a line perpendicular to lineSolutionMelanie computes the mean , the median, and the modes of the values that are the dates in the months of . Thus her data consist of , , . . . , , , , and . Let be the median of the modes. Which of the following statements is true?SolutionProblem 2Problem 3Problem 4Problem 5Problem 6Problem 7For a set of four distinct lines in a plane, there are exactly distinct points that lie on two or more of the lines. What is the sum of allpossible values of ?Solution A sequence of numbers is deﬁned recursively by, , and for all . Then can be written as , whereandare relatively prime positive inegers. What is Solution The ﬁgure below shows circles of radius within a larger circle. All the intersections occur at points of tangency. What is the area ofthe region, shaded in the ﬁgure, inside the larger circle but outside all the circles of radius?Solution For some positive integer , the repeating base- representation of the (base-ten) fraction is. What is ?Solution Positive real numbers and satisfy and. What is ?Problem 8Problem 9Problem 10Problem 11Problem 12Solution How many ways are there to paint each of the integers either red, green, or blue so that each number has a different colorfrom each of its proper divisors?Solution For a certain complex number, the polynomial has exactly 4 distinct roots. What is?Solution Positive real numbers andhave the property that and all four terms on the left are positive integers, where denotes the base- logarithm. What is?Solution The numbers are randomly placed into the squares of a grid. Each square gets one number, and each of thenumbers is used once. What is the probability that the sum of the numbers in each row and each column is odd?Solution Let denote the sum of the th powers of the roots of the polynomial . In particular, ,, and . Let , , and be real numbers such that for ,, What is?Solution A sphere with center has radius . A triangle with sides of length and is situated in space so that each of its sides is tangent to the sphere. What is the distance between and the plane determined by the triangle?SolutionIn with integer side lengths,What is the least possible perimeter for ?Problem 13Problem 14Problem 15Problem 16Problem 17Problem 18Problem 19Real numbers between and , inclusive, are chosen in the following manner. A fair coin is ﬂipped. If it lands heads, then it is ﬂipped again and the chosen number is if the second ﬂip is heads and if the second ﬂip is tails. On the other hand, if the ﬁrst coin ﬂip is tails, then the number is chosen uniformly at random from the closed interval . Two random numbersandare chosen independently in thismanner. What is the probability that?Solution Let What is Solution Circles and , both centered at , have radii and , respectively. Equilateral triangle , whose interior lies in the interior of but in the exterior of , has vertex on , and the line containing side is tangent to . Segments and intersect at ,and . Thencan be written in the form for positive integers ,, , with . What is ?Solution Deﬁne binary operations andby for all real numbers and for which these expressions are deﬁned. The sequence is deﬁned recursively byand for all integers . To the nearest integer, what is ?SolutionFor how many integers between and , inclusive, isan integer? (Recall that .)Problem 21Problem 22Problem 23Problem 24Copyright © 2019 Art of Problem SolvingLet be a triangle whose angle measures are exactly , , and . For each positive integer deﬁne to be the foot of the altitude from to line . Likewise, deﬁne to be the foot of the altitude from to line, and to be the foot of the altitude from to line. What is the least positive integer for whichis obtuse?Solution2019 AMC 12A (Problems • Answer Key • Resources (/Forum/resources.php?c=182&cid=44&year=2019))Preceded by2018 AMC 12B Problems Followed by 2019 AMC 12B Problems1 •2 •3 •4 •5 •6 •7 •8 •9 • 10 • 11 • 12 • 13 • 14 • 15 • 16 • 17 • 18• 19 • 20 • 21 • 22 • 23 • 24 • 25All AMC 12 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America ()'s American Mathematics Competitions ().Retrieved from "https:///wiki/index.php?title=2019_AMC_12A_Problems&oldid=101818"See also2019 AMC 12A Answer Key1. E2. D3. B4. D5. C6. C7. E8. D9. E10. A11. D12. B13. E14. E15. D16. B17. D18. D19. A20. B21. C22. E23. D24. D25. E2019 AMC 12B Answer Key1. D2. E3. E4. C5. B6. A7. A8. A9. B10. E11. D12. D13. C14. C15. E16. A17. D18. C19. B20. C21. B22. C23. C24. C25. C2018 AMC 12A ProblemsA large urn contains balls, of which are red and the rest are blue. How many of the blue balls must be removed so that the percentage of red balls in the urn will be ? (No red balls are to be removed.)SolutionWhile exploring a cave, Carl comes across a collection of -pound rocks worth each, -pound rocks worth each, and -pound rocks worth each. There are at least of each size. He can carry at most pounds. What is the maximum value, in dollars, of the rocks he can carry out of the cave?SolutionSolutionAlice, Bob, and Charlie were on a hike and were wondering how far away the nearest town was. When Alice said, "We are at least 6 miles away," Bob replied, "We are at most 5 miles away." Charlie then remarked, "Actually the nearest town is at most 4 miles away." It turned out that none of the three statements were true. Letbe the distance in miles to the nearest town. Which of the following intervals is the set of all possible values of ?Solution What is the sum of all possible values of for which the polynomials andhave a root in common?Solution For positive integers andsuch that , both the mean and the median of the set are equalto . What is ?SolutionFor how many (not necessarily positive) integer values of is the value of an integer?SolutionAll of the triangles in the diagram below are similar to iscoceles triangle , in which . Each of the 7 smallest triangles has area 1, andhas area 40. What is the area of trapezoid ?Solution Which of the following describes the largest subset of values ofwithin the closed interval for which for everybetweenand , inclusive?Solution How many ordered pairs of real numbers satisfy the following system of equations?SolutionA paper triangle with sides of lengths 3,4, and 5 inches, as shown, is folded so that point falls on point . What is the length in inches of the crease?Problem 5Problem 6Problem 7Problem 8Problem 9Problem 10Problem 11Solution Let be a set of 6 integers taken fromwith the property that ifand are elements of with , thenis not a multiple of . What is the least possible value of an element in Solution How many nonnegative integers can be written in the form where for ?Solution The solutions to the equation, where is a positive real number other than or , can be written as where and are relatively primepositive integers. What is ?Solution A scanning code consists of a grid of squares, with some of its squares colored black and the rest colored white. There must be at least one square of each color in this grid of squares. A scanning code is called if its look does not change when the entire square is rotated by a multiple of counterclockwise around its center, nor when it is reflected across a line joining opposite corners or a line joining midpoints of opposite sides. What is the totalnumber of possible symmetric scanning codes?Solution Which of the following describes the set of values of for which the curvesand in the real -plane intersect at exactly points?SolutionFarmer Pythagoras has a field in the shape of a right triangle. The right triangle's legs have lengths 3 and 4 units. In the corner where those sides meet at a right angle, he leaves a small unplanted square so that from the air it looks like the right angle symbol. The rest of the field is planted. The shortest distance from to the hypotenuse is 2 units. What fraction of the field is planted?Problem 12Problem 13Problem 14Problem 15Problem 16Problem 17SolutionTriangle with and has area . Let be the midpoint of, and let be the midpoint of . The angle bisector of intersects andat and , respectively. What is the area of quadrilateral ?Solution Letbe the set of positive integers that have no prime factors other than , , or . The infinite sum of the reciprocals of the elements of can be expressed as , where and are relatively prime positive integers. What is ?Solution Triangle is an isosceles right triangle with . Let be the midpoint of hypotenuse . Points and lie on sidesand ,respectively, so that andis a cyclic quadrilateral. Given that triangle has area , the length can be written as , where ,, and are positive integers andis not divisible by the square of any prime. What is the value of?Solution Which of the following polynomials has the greatest real root?Solution The solutions to the equations and where form the vertices of a parallelogram in the complex plane. The areaof this parallelogram can be written in the formwhereand are positive integers and neither nor is divisible by the square of any prime number. What is Solution In and Points andlie on sides and respectively, so that Let andbe the midpoints of segments and respectively. What is the degree measure of the acute angle formed by lines and Solution Alice, Bob, and Carol play a game in which each of them chooses a real number between 0 and 1. The winner of the game is the one whose number is between the numbers chosen by the other two players. Alice announces that she will choose her number uniformly at random from all the numbers between 0 and 1, and Bob announces that he will choose his number uniformly at random from all the numbers between and Armed with this information, what number should Carol choose to maximize her chance of winning? Solution For a positive integer and nonzero digits , , and , let be the -digit integer each of whose digits is equal to ; let be the-digit integer each of whose digits is equal to , and let be the -digit (not -digit) integer each of whose digits is equal to . What is the greatest possible value offorwhich there are at least two values of such that ?SolutionProblem 18Problem 19Problem 20Problem 21Problem 22Problem 23Problem 24Problem 25See also2018 AMC 12A (Problems • Answer Key • Resources)Preceded by 2017 AMC 12B ProblemsFollowed by 2018 AMC 12B Problems1 ·2 ·3 ·4 ·5 ·6 ·7 ·8 ·9 · 10 · 11 · 12 · 13 · 14 · 15 · 16 · 17 · 18 · 19 · 20 · 21 · 22 · 23 · 24 · 25All AMC 12 Problems and SolutionsThe problems on this page are copyrighted by the Mathematical Association of America's American Mathematics Competitions.Retrieved from "/wiki/index.php?title=2018_AMC_12A_Problems&oldid=94197"Category: AMC 12 ProblemsCopyright © 2018 Art of Problem Solving2018 AMC 12A Answer Key1. D2. C3. E4. D5. E6. B7. E8. E9. E10. C11. D12. C13. D14. D15. B16. E17. D18. D19. C20. D21. B22. A23. E24. B25. DRetrieved from "/wiki/index.php?title=2018_AMC_12A_Answer_Key&oldid=90552"Copyright © 2018 Art of Problem Solving2018 AMC 12B ProblemsKate bakes 20-inch by 18-inch pan of cornbread. The cornbread is cut into pieces that measure 2 inches by 2 inches.How many pieces of cornbread does the pan contain?SolutionSam drove 96 miles in 90 minutes. His average speed during the first 30 minutes was 60 mph (miles per hour), and his average speed during the second 30 minutes was 65 mph. What was his average speed, in mph, during the last 30minutes?Solution A line with slope 2 intersects a line with slope 6 at the point . What is the distance between the -intercepts ofthese two lines? Solution A circle has a chord of length , and the distance from the center of the circle to the chord is . What is the area of thecircle?Solution How many subsets ofcontain at least one prime number?Solution Suppose cans of soda can be purchased from a vending machine for quarters. Which of the following expressionsdescribes the number of cans of soda that can be purchased fordollars, where 1 dollar is worth 4 quarters?SolutionWhat is the value ofSolutionProblem 2Problem 3Problem 4Problem 5Problem 6Problem 7Problem 8Line segment is a diameter of a circle with . Point, not equal toor, lies on the circle. As point moves around the circle, the centroid (center of mass) of traces out a closed curve missing two points. To the nearest positive integer, what is the area of the region bounded by this curve?SolutionWhat isSolution A list of positive integers has a unique mode, which occurs exactly times. What is the least number of distinct values that can occur in the list?SolutionA closed box with a square base is to be wrapped with a square sheet of wrapping paper. The box is centered on the wrapping paper with the vertices of the base lying on the midlines of the square sheet of paper, as shown in the figureon the left. The four corners of the wrapping paper are to be folded up over the sides and brought together to meet at the center of the top of the box, point in the figure on the right. The box has base length and height . What is the area of the sheet of wrapping paper?Solution Side of has length . The bisector of angle meets at , and . The set of all possiblevalues of is an open interval . What is ?Problem 9Problem 10Problem 11Problem 12Solution Square has side length . Point lies inside the square so thatand . The centroids of, ,, and are the vertices of a convex quadrilateral. What is the area of that quadrilateral?Solution Joey, Chloe, and their daughter Zoe all have the same birthday. Joey is 1 year older than Chloe, and Zoe is exactly 1 year old today. Today is the first of the 9 birthdays on which Chloe's age will be an integral multiple of Zoe's age. What will be the sum of the two digits of Joey's age the next time his age is a multiple of Zoe's age?SolutionHow many odd positive 3-digit integers are divisible by 3 but do not contain the digit 3?Solution The solutions to the equation are connected in the complex plane to form a convex regular polygon,three of whose vertices are labeled and . What is the least possible area ofSolutionLet and be positive integers such thatand is as small as possible. What is ?Problem 13Problem 14Problem 15Problem 16Problem 17。

美国数学竞赛AMC题目及答案

2.3.Whatisthevalueof4.5.Hammieisinthegradeandweighs106pounds.Hisquadrupletsistersaretinybabiesandweigh5,5,6,and8pounds.W hichisgreater,theaverage(mean)weightofthesefivechildrenorthemedianweight,andbyhowman ypounds6.Thenumberineachboxbelowistheproductofthenumbersinthetwoboxesthattouchitintherowab ove.Forexample,.Whatisthemissingnumberinthetoprow7.8.Afaircoinistossed3times.Whatistheprobabilityofatleasttwoconsecutiveheads9.TheIncredibleHulkcandoublethedistancehejumpswitheachsucceedingjump.Ifhisfirstjumpis1 meter,thesecondjumpis2meters,thethirdjumpis4meters,andsoon,thenonwhichjumpwillhefirst beabletojumpmorethan1kilometer10.Whatistheratiooftheleastcommonmultipleof180and594tothegreatestcommonfactorof180a nd59411.12.Atthe2013WinnebagoCountyFairavendorisofferinga"fairspecial"onsandals.Ifyoubuyonepai rofsandalsattheregularpriceof$50,yougetasecondpairata40%discount,andathirdpairathalfthe regularprice.Javiertookadvantageofthe"fairspecial"tobuythreepairsofsandals.Whatpercentag eofthe$150regularpricedidhesave13.WhenClaratotaledherscores,sheinadvertentlyreversedtheunitsdigitandthetensdigitofones core.Bywhichofthefollowingmightherincorrectsumhavedifferedfromthecorrectone14.Letthetwodigitsbe and.Thecorrectscorewas.Claramisinterpreteditas.Thedifferencebetweenthetwoiswhichfactorsinto.Therefore,sincethedifferenceisamultipleof9,theonlyanswerchoicethatisamultipleof9 is.15.If,,and,whatistheproductof,,and16.AnumberofstudentsfromFibonacciMiddleSchoolaretakingpartinacommunityserviceproject.Theratioof-gradersto-gradersis,andthetheratioof-gradersto-gradersis .Whatisthesmallestnumberofstudentsthatcouldbeparticipatingintheproject17.Thesumofsixconsecutivepositiveintegersis2013.Whatisthelargestofthesesixintegers18.--Arpanliku16:22,27November2013(EST)Courtesyof19.Bridget,Cassie,andHannaharediscussingtheresultsoftheirlastmathtest.HannahshowsBridg etandCassiehertest,butBridgetandCassiedon'tshowtheirstoanyone.Cassiesays,'Ididn'tgetthel owestscoreinourclass,'andBridgetadds,'Ididn'tgetthehighestscore.'Whatistherankingofthethr eegirlsfromhighesttolowest20.Arectangleisinscribedinasemicirclewithlongersideonthediameter.Whatistheareaofthesemicircle21.22.Toothpicksareusedtomakeagridthatis60toothpickslongand32toothpickswide.Howmanytoo thpicksareusedaltogether23.Angle of isarightangle.Thesidesofarethediametersofsemicirclesasshown.Theareaofthesemicircleon equals,andthearcofthesemicircleon haslength.Whatistheradiusofthesemicircleon24.Squares,,and areequalinarea.Points andarethemidpointsofsides and,respectively.Whatistheratiooftheareaoftheshadedpentagontothesumoftheareasofthethreesquares25.Aballwithdiameter4inchesstartsatpointAtorollalongthetrackshown.Thetrackiscomprisedof3semicirculararcswhoseradiiare inches,inches,and1.2.The50%offpriceofhalfapoundoffishis$3,sothe100%,ortheregularprice,ofahalfpoundoffishis$6.Consequently,ifhalfapoundoffishcosts$6,thenawholepoundoffishis dollars.3.Noticethatwecanpairupeverytwonumberstomakeasumof1:Therefore,theansweris.4.Eachofhersevenfriendspaid tocoverJudi'sportion.Therefore,Judi'sportionmustbe.SinceJudiwassupposedtopay ofthetotalbill,thetotalbillmustbe.5.Themedianhereisobviouslylessthanthemean,sooption(A)and(B)areout. Liningupthenumbers(5,5,6,8,106),weseethatthemedianweightis6pounds.Theaverageweightofthefivekidsis.Therefore,theaverageweightisbigger,by pounds,makingtheanswer.6.Solution1:WorkingBackwardsLetthevalueintheemptyboxinthemiddlerowbe,andthevalueintheemptyboxinthetoprowbe. istheanswerwe'relookingfor.Weseethat,making.Itfollowsthat,so.Solution2:JumpingBacktotheStartAnotherwaytodothisproblemistorealizewhatmakesupthebottommostnumber.Thismethoddoes n'tworkquiteaswellforthisproblem,butinalargertree,itmightbefaster.(Inthiscase,Solution1wou ldbefastersincethere'sonlytwomissingnumbers.)Again,letthevalueintheemptyboxinthemiddlerowbe,andthevalueintheemptyboxinthetoprowbe.istheanswerwe'relookingfor. Wecanwritesomeequations: Nowwecansubstituteintothefirstequationusingthetwoothers:7.IfTreysaw,thenhesaw.2minutesand45secondscanalsobeexpressedas seconds.Trey'srateofseeingcars,,canbemultipliedbyonthetopandbottom(andpreservethesamerate):.Itfollowsthatthemostlikelynumberofcarsis. Solution2minutesand secondsisequalto.SinceTreyprobablycountsaround carsevery seconds,thereare groupsofcarsthatTreymostlikelycounts.Since,theclosestanswerchoiceis.8.First,thereare waystoflipthecoins,inorder. ThewaystogettwoconsecutiveheadsareHHT andTHH. ThewaytogetthreeconsecutiveheadsisHHH.Therefore,theprobabilityofflippingatleasttwoconsecutiveheadsis.9.Thisisageometricsequenceinwhichthecommonratiois2.Tofindthejumpthatwouldbeovera1000meters,wenotethat.However,becausethefirsttermis andnot,thesolutiontotheproblemis10.TofindeithertheLCMortheGCFoftwonumbers,alwaysprimefactorizefirst. Theprimefactorizationof.Theprimefactorizationof.Then,findthegreatestpowerofallthenumbersthereare;ifonenumberisonebutnottheother,useit(t hisis).Multiplyallofthesetoget5940.FortheGCFof180and594,usetheleastpowerofallofthenumbersthatareinbothfactorizationsandmultiply.=18.Thustheanswer==. Westartoffwithasimilarapproachastheoriginalsolution.Fromtheprimefactorizations,theGCFis .Itisawellknownfactthat.Sowehave,.Dividingby yields.Therefore,.11.Weusethatfactthat.Letd=distance,r=rateorspeed,andt=time.Inthiscase,letrepresentthetime.OnMonday,hewasatarateof.So,.ForWednesday,hewalkedatarateof.Therefore,.OnFriday,hewalkedatarateof.So,.Addingupthehoursyields++=.WenowfindtheamountoftimeGrandfatherwouldhavetakenifhewalkedatperday.Setuptheequation,.Tofindtheamountoftimesaved,subtractthetwoamounts:-=.Toconvertthistominutes,wemultiplyby.Thus,thesolutiontothisproblemis12.First,findtheamountofmoneyonewillpayforthreesandalswithoutthediscount.Wehave.Then,findtheamountofmoneyusingthediscount:.Findingthepercentageyields.Tofindthepercentsaved,wehave13.Letthetwodigitsbe and.Thecorrectscorewas.Claramisinterpreteditas.Thedifferencebetweenthetwoiswhichfactorsinto.Therefore,sincethedifferenceisamultipleof9,theonlyanswerchoicethatisamultipleof9is.14.Theprobabilitythatbothshowagreenbeanis.Theprobabilitythatbothshowaredbeanis.Thereforetheprobabilityis15.Therefore,.Therefore,.Tomostpeople,itwouldnotbeimmediatelyevidentthat,sowecanmultiply6'suntilwegetthedesirednumber:,so.Thereforetheansweris.16.Solution1:AlgebraWemultiplythefirstratioby8onbothsides,andthesecondratioby5togetthesamenumberfor8thgra ders,inorderthatwecanputthetworatiostogether:Therefore,theratioof8thgradersto7thgradersto6thgradersis.Sincetheratioisinlowestterms,thesmallestnumberofstudentsparticipatingintheprojectis.Solution2:FakesolvingThenumberof8thgradershastobeamultipleof8and5,soassumeitis40(thesmallestpossibility).Thenthereare6thgradersand7thgraders.Thenumbersofstudentsis17.Solution1Themeanofthesenumbersis.Thereforethenumbersare,sotheanswerisSolution2Letthe numberbe.Thenourdesirednumberis.Ourintegersare,sowehavethat.Solution3Letthefirsttermbe.Ourintegersare.Wehave,18.Solution1Therearecubesonthebaseofthebox.Then,foreachofthe4layersabovethebottom(assinceeachcubeis1foot by1footby1footandtheboxis5feettall,thereare4feetleft),therearecubes.Hence,theansweris.Solution2Wecanjustcalculatethevolumeoftheprismthatwascutoutoftheoriginalbox.Eachinteriorsideofthefortwillbe feetshorterthaneachsideoftheoutside.Sincethefloorisfoot,theheightwillbe feet.Sothevolumeoftheinteriorboxis.Thevolumeoftheoriginalboxis.Therefore,thenumberofblockscontainedinthefortis.19.IfHannahdidbetterthanCassie,therewouldbenowayshecouldknowforsurethatshedidn'tgett helowestscoreintheclass.Therefore,HannahdidworsethanCassie.Similarly,ifHannahdidworseth anBridget,thereisnowayBridgetcouldhaveknownthatshedidn'tgetthehighestintheclass.Therefore,HannahdidbetterthanBridget,soourorderis.20.Asemicirclehassymmetry,sothecenterisexactlyatthemidpointofthe2sideontherectangle,makingtheradius,bythePythagoreanTheorem,.Theareais.21.ThenumberofwaystogetfromSamantha'shousetoCityParkis,andthenumberofwaystogetfromCityParktoschoolis.Sincethere'sonewaytogothroughCityPark(justwalkingstraightthrough),thenumberofdifferentwaystogofromSamantha'shousetoCityParktoschool.22.Thereare verticalcolumnswithalengthof toothpicks,andthereare horizontalrowswithalengthof toothpicks.Aneffectivewaytoverifythisistotryasmallcase,i.e.agridoftoothpicks.Thus,ouransweris.23.Solution1.Solution2WegoasinSolution1,findingthediameterofthecircleonACandAB.Then,anextendedversionofthet heoremsaysthatthesumofthesemicirclesontheleftisequaltothebiggestone,sotheareaofthelargestis,andthemiddleoneis,sotheradiusis.24.Firstlet(where isthesidelengthofthesquares)forsimplicity.Wecanextenduntilithitstheextensionof.Callthispoint.Theareaoftriangle thenis Theareaofrectangle is.Thus,ourdesiredareais.Now,theratiooftheshadedareatothecombinedareaofthethreesquaresis.Solution2Letthesidelengthofeachsquarebe.Lettheintersectionof and be.Since,.Since andareverticalangles,theyarecongruent.Wealsohave bydefinition.Sowehave by congruence.Therefore,.Since and aremidpointsofsides,.Thiscombinedwith yields.Theareaoftrapezoid is.Theareaoftriangle is.Sotheareaofthepentagon is.Theareaofthe squaresis.Therefore,.Solution3Lettheintersectionof and be.Nowwehave and.Becausebothtriangleshasasideoncongruentsquarestherefore.Because and areverticalangles.Alsoboth and arerightanglesso.ThereforebyAAS(Angle,Angle,Side).Thentranslating/rotatingtheshaded intothepositionofSotheshadedareanowcompletelycoversthesquareSettheareaofasquareasTherefore,.25.Solution1Theradiusoftheballis2inches.Ifyouthinkabouttheballrollingordrawapathfortheball(seefigurebelow),youseethatinAandCitloses inches,anditgains inchesonB.So,thedeparturefromthelengthofthetrackmeansthattheansweris.Solution2Thetotallengthofallofthearcsis.Sincewewantthepathfromthecenter,theactualdistancewillbeshorter.Therefore,theonlyanswerchoicelessthan is.Thissolutionmaybeinvalidbecausetheactualdistancecanbelongerifthepaththecent ertravelsisontheoutsideofthecurve,asitisinthemiddlebump.。

美国数学竞赛AMC题目及答案

2.3.What is the value of ?4.Eight friends ate at a restaurant and agreed to share the bill equally. Because Judi forgot her money, each of her seven friends paid an extra $2.50 to cover her portion of the total bill. What was the total bill?5.Hammie is in the grade and weighs 106 pounds. His quadruplet sisters are tiny babies and weigh 5, 5, 6, and 8 pounds. Which is greater, the average (mean) weight of these five children or the median weight, and by how many pounds?6.The number in each box below is the product of the numbers in the two boxes that touch it in the row above. For example, . What is the missing number in the top row?7.Trey and his mom stopped at a railroad crossing to let a train pass. As the train began to pass, Trey counted 6 cars in the first 10 seconds. It took the train 2 minutes and 45 seconds to clear the crossing at a constant speed. Which of the following was the most likely number of cars in the train?8.A fair coin is tossed 3 times. What is the probability of at least two consecutive heads?9.The Incredible Hulk can double the distance he jumps with each succeeding jump. If his first jump is 1 meter, the second jump is 2 meters, the third jump is 4 meters, and so on, then on which jump will he first be able to jump more than 1 kilometer?10.What is the ratio of the least common multiple of 180 and 594 to the greatest common factor of 180 and 594?11. Ted's grandfather used his treadmill on 3 days this week. He went 2 miles each day. On Monday he jogged at a speed of 5 miles per hour. He walked at the rate of 3 miles per hour on Wednesday and at 4 miles per hour on Friday. If Grandfather had always walked at 4 miles per hour, he would have spent less time on the treadmill. How many minutes less?12. At the 2013 Winnebago County Fair a vendor is offering a "fair special" on sandals. If you buy one pair of sandals at the regular price of $50, you get a second pair at a 40% discount, and a third pair at half the regular price. Javier took advantage of the "fair special" to buy three pairs of sandals. What percentage of the $150 regular price did he save?13. When Clara totaled her scores, she inadvertently reversed the units digit and the tens digit of one score. By which of the following might her incorrect sum have differed from the correct one?14. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .15. If , , and , what is the product of , , and ?16. A number of students from Fibonacci Middle School are taking part in a community service project. The ratio of -graders to -graders is , and the the ratio of -graders to -graders is . What is the smallest number of students that could be participating in the project?17. The sum of six consecutive positive integers is 2013. What is the largest of these six integers?18. Isabella uses one-foot cubical blocks to build a rectangular fort that is 12 feet long, 10 feet wide, and 5 feet high. The floor and the four walls are all one foot thick. How many blocks does the fort contain?--Arpanliku 16:22, 27 November 2013 (EST) Courtesy of Lord.of.AMC19. Bridget, Cassie, and Hannah are discussing the results of their last math test. Hannah shows Bridget and Cassie her test, but Bridget and Cassie don't show theirs to anyone. Cassie says, 'I didn't get the lowest score in our class,' and Bridget adds, 'I didn't get the highest score.' What is the ranking of the three girls from highest to lowest?20. A rectangle is inscribed in a semicircle with longer side on the diameter. What is the area of the semicircle?21. Samantha lives 2 blocks west and 1 block south of the southwest corner of City Park. Her school is 2 blocks east and 2 blocks north of the northeast corner of City Park. On school days she bikes on streets to the southwest corner of City Park, then takes a diagonal path through the park to the northeast corner, and then bikes on streets to school. If her route is as short as possible, how many different routes can she take?22. Toothpicks are used to make a grid that is 60 toothpicks long and 32 toothpicks wide. How many toothpicks are used altogether?23. Angle of is a right angle. The sides of are the diameters of semicircles as shown. The area of the semicircle on equals , and the arc of the semicircle on has length .What is the radius of the semicircle on ?24. Squares , , and are equal in area. Points and are the midpoints of sidesand , respectively. What is the ratio of the area of the shaded pentagon to the sum of the areas of the three squares?25. A ball with diameter 4 inches starts at point A to roll along the track shown. The track is comprised of 3 semicircular arcs whose radii are inches, inches, and inches, respectively. The ball always remains in contact with the track and does not slip. What is the distance the center of theball travels over the course from A to B?1.2.The 50% off price of half a pound of fish is $3, so the 100%, or the regular price, of a half pound of fish is $6. Consequently, if half a pound of fish costs $6, then a whole pound of fish is dollars.3.Notice that we can pair up every two numbers to make a sum of 1:Therefore, the answer is .4.Each of her seven friends paid to cover Judi's portion. Therefore, Judi's portion must be . Since Judi was supposed to pay of the total bill, the total bill must be .5.The median here is obviously less than the mean, so option (A) and (B) are out.Lining up the numbers (5, 5, 6, 8, 106), we see that the median weight is 6 pounds.The average weight of the five kids is .Therefore, the average weight is bigger, by pounds, making the answer.6.Solution 1: Working BackwardsLet the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We see that , making .It follows that , so .Solution 2: Jumping Back to the StartAnother way to do this problem is to realize what makes up the bottommost number. This method doesn't work quite as well for this problem, but in a larger tree, it might be faster. (In this case, Solution 1 would be faster since there's only two missing numbers.)Again, let the value in the empty box in the middle row be , and the value in the empty box in the top row be . is the answer we're looking for.We can write some equations:Now we can substitute into the first equation using the two others:7.If Trey saw , then he saw .2 minutes and 45 seconds can also be expressed as seconds.Trey's rate of seeing cars, , can be multiplied by on the top and bottom (and preserve the same rate):. It follows that the most likely number of cars is . Solution 2minutes and seconds is equal to .Since Trey probably counts around cars every seconds, there are groups of cars that Trey most likely counts. Since , the closest answer choice is .8.First, there are ways to flip the coins, in order.The ways to get two consecutive heads are HHT and THH.The way to get three consecutive heads is HHH.Therefore, the probability of flipping at least two consecutive heads is .9.This is a geometric sequence in which the common ratio is 2. To find the jump that would be over a 1000 meters, we note that .However, because the first term is and not , the solution to the problem is10. To find either the LCM or the GCF of two numbers, always prime factorize first.The prime factorization of .The prime factorization of .Then, find the greatest power of all the numbers there are; if one number is one but not the other, use it (this is ). Multiply all of these to get 5940.For the GCF of 180 and 594, use the least power of all of the numbers that are in both factorizations and multiply. = 18.Thus the answer = = .We start off with a similar approach as the original solution. From the prime factorizations, the GCF is .It is a well known fact that . So we have,.Dividing by yields .Therefore, .11. We use that fact that . Let d= distance, r= rate or speed, and t=time. In this case, let represent the time.On Monday, he was at a rate of . So, .For Wednesday, he walked at a rate of . Therefore, .On Friday, he walked at a rate of . So, .Adding up the hours yields + + = .We now find the amount of time Grandfather would have taken if he walked at per day. Set up the equation, .To find the amount of time saved, subtract the two amounts: - = . To convert this to minutes, we multiply by .Thus, the solution to this problem is12. First, find the amount of money one will pay for three sandals without the discount. We have.Then, find the amount of money using the discount: .Finding the percentage yields .To find the percent saved, we have13. Let the two digits be and .The correct score was . Clara misinterpreted it as . The difference between the two is which factors into . Therefore, since the difference is a multiple of 9, the only answerchoice that is a multiple of 9 is .14. The probability that both show a green bean is . The probability that both show a red bean is . Therefore the probability is15.Therefore, .Therefore, .To most people, it would not be immediately evident that , so we can multiply 6's until we get the desired number:, so .Therefore the answer is .16. Solution 1: AlgebraWe multiply the first ratio by 8 on both sides, and the second ratio by 5 to get the same number for 8th graders, in order that we can put the two ratios together:Therefore, the ratio of 8th graders to 7th graders to 6th graders is . Since the ratio is in lowest terms, the smallest number of students participating in the project is .Solution 2: FakesolvingThe number of 8th graders has to be a multiple of 8 and 5, so assume it is 40 (the smallest possibility). Then there are 6th graders and 7th graders. The numbers of students is17. Solution 1The mean of these numbers is . Therefore the numbers are, so the answer isSolution 2Let the number be . Then our desired number is .Our integers are , so we have that.Solution 3Let the first term be . Our integers are . We have,18. Solution 1There are cubes on the base of the box. Then, for each of the 4 layers above the bottom (as since each cube is 1 foot by 1 foot by 1 foot and the box is 5 feet tall, there are 4 feet left), there arecubes. Hence, the answer is .Solution 2We can just calculate the volume of the prism that was cut out of the original box. Each interior side of the fort will be feet shorter than each side of the outside. Since the floor is foot, the height will be feet. So the volume of the interior box is .The volume of the original box is . Therefore, the number of blocks contained inthe fort is .19. If Hannah did better than Cassie, there would be no way she could know for sure that she didn't get the lowest score in the class. Therefore, Hannah did worse than Cassie. Similarly, if Hannah did worse than Bridget, there is no way Bridget could have known that she didn't get the highest in the class.Therefore, Hannah did better than Bridget, so our order is .20.A semicircle has symmetry, so the center is exactly at the midpoint of the 2 side on the rectangle, making the radius, by the Pythagorean Theorem, . The area is .21.The number of ways to get from Samantha's house to City Park is , and the number of ways toget from City Park to school is . Since there's one way to go through City Park (just walking straight through), the number of different ways to go from Samantha's house to City Park to school .22. There are vertical columns with a length of toothpicks, and there are horizontal rows with a length of toothpicks. An effective way to verify this is to try a small case, i.e. a grid of toothpicks.Thus, our answer is .23. Solution 1If the semicircle on AB were a full circle, the area would be 16pi. Therefore the diameter of the first circle is 8. The arc of the largest semicircle would normally have a complete diameter of 17. The Pythagoreantheorem says that the other side has length 15, so the radius is .Solution 2We go as in Solution 1, finding the diameter of the circle on AC and AB. Then, an extended version of the theorem says that the sum of the semicircles on the left is equal to the biggest one, so the area of thelargest is , and the middle one is , so the radius is .24.First let (where is the side length of the squares) for simplicity. We can extend until it hits theextension of . Call this point . The area of triangle then is The area of rectangle is . Thus, our desired area is . Now, the ratio of the shaded area to thecombined area of the three squares is .Let the side length of each square be .Let the intersection of and be .Since , . Since and are vertical angles, they are congruent. We also have by definition.So we have by congruence. Therefore, .Since and are midpoints of sides, . This combined with yields.The area of trapezoid is .The area of triangle is .So the area of the pentagon is .The area of the squares is .Therefore, .Let the intersection of and be .Now we have and .Because both triangles has a side on congruent squares therefore .Because and are vertical angles .Also both and are right angles so .Therefore by AAS(Angle, Angle, Side) .Then translating/rotating the shaded into the position ofSo the shaded area now completely covers the squareSet the area of a square asTherefore, .25. Solution 1The radius of the ball is 2 inches. If you think about the ball rolling or draw a path for the ball (see figure below), you see that in A and C it loses inches, and it gains inches on B.So, the departure from the length of the track means that the answer is . Solution 2The total length of all of the arcs is . Since we want the path from the center, the actual distance will be shorter. Therefore, the only answer choice less than is . Thissolution may be invalid because the actual distance can be longer if the path the center travels is on the outside of the curve, as it is in the middle bump.古希腊哲学大师亚里士多德说：人有两种，一种即“吃饭是为了活着”,一种是“活着是为了吃饭”.一个人之所以伟大，首先是因为他有超于常人的心。

2018年AMC8考题和答案解析

2017 AMC 8 考题及答案Problem 1Which of the following values is largestProblem 2Alicia, Brenda, and Colby were the candidates in a recent election for student president. The pie chart below shows how the votes were distributed among the three candidates. If Brenda received 36 votes, then how many votes were cast all togetherProblem 3What is the value of the expressionProblem 4When 0.000315 is multiplied by 7,928,564 the product is closest to which of the followingProblem 5What is the value of the expressionProblem 6If the degree measures of the angles of a triangle are in the ratio , what is the degree measure of the largest angle of the triangle Problem 7Let be a 6-digit positive integer, such as 247247, whose first three digits are the same as its last three digits taken in the same order. Which of the following numbers must also be a factor ofProblem 8Malcolm wants to visit Isabella after school today and knows the street where she lives but doesn't know her house number. She tells him, "My house number has two digits, and exactly three of the following four statements about it are true."(1) It is prime.(2) It is even.(3) It is divisible by 7.(4) One of its digits is 9.This information allows Malcolm to determine Isabella's house number. What is its units digitProblem 9All of Marcy's marbles are blue, red, green, or yellow. One third of her marbles are blue, one fourth of them are red, and six of them are green. What is the smallest number of yellow marbles that Marcy could have Problem 10A box contains five cards, numbered 1, 2, 3, 4, and 5. Three cards are selected randomly without replacement from the box. What is the probability that 4 is the largest value selectedProblem 11A square-shaped floor is covered with congruent square tiles. If the total number of tiles that lie on the two diagonals is 37, how many tiles cover the floorProblem 12The smallest positive integer greater than 1 that leaves a remainder of 1 when divided by 4, 5, and 6 lies between which of the following pairs of numbersProblem 13Peter, Emma, and Kyler played chess with each other. Peter won 4 games and lost 2 games. Emma won 3 games and lost 3 games. If Kyler lost 3 games, how many games did he winProblem 14Chloe and Zoe are both students in Ms. Demeanor's math class. Last night they each solved half of the problems in their homework assignment alone and then solved the other half together. Chloe had correct answers to only of the problems she solved alone, but overall of her answers were correct. Zoe had correct answers to of the problems she solved alone. What was Zoe's overall percentage of correct answersProblem 15In the arrangement of letters and numerals below, by how many different paths can one spell AMC8 Beginning at the A in the middle, a path allows only moves from one letter to an adjacent (above, below, left, or right, but not diagonal) letter. One example of such a path is traced in the picture.Problem 16In the figure below, choose point on so that and have equal perimeters. What is the area ofProblem 17Starting with some gold coins and some empty treasure chests, I tried to put 9 gold coins in each treasure chest, but that left 2 treasure chestsempty. So instead I put 6 gold coins in each treasure chest, but then I had 3 gold coins left over. How many gold coins did I have Problem 18In the non-convex quadrilateral shown below, is a right angle, , , , and .What is the area of quadrilateralProblem 19For any positive integer , the notation denotes the product of the integers through . What is the largest integer for which is a factor of the sumProblem 20An integer between and , inclusive, is chosen at random. What is the probability that it is an odd integer whose digits are all distinctProblem 21Suppose , , and are nonzero real numbers, and . What are the possible value(s) forProblem 22In the right triangle , , , and angle is a right angle. A semicircle is inscribed in the triangle as shown. What is the radius of the semicircleProblem 23Each day for four days, Linda traveled for one hour at a speed that resulted in her traveling one mile in an integer number of minutes. Each day after the first, her speed decreased so that the number of minutes to travel one mile increased by 5 minutes over the preceding day. Each of the four days, her distance traveled was also an integer number of miles. What was the total number of miles for the four tripsProblem 24Mrs. Sanders has three grandchildren, who call her regularly. One calls her every three days, one calls her every four days, and one calls her every five days. All three called her on December 31, 2016. On how many days during the next year did she not receive a phone call from any of her grandchildrenProblem 25In the figure shown, and are line segments each of length 2, and . Arcs and are each one-sixth of a circle with radius 2. What is the area of the region shown2017 AMC 8 Answer Key1. A2. E3. C4. D5. B6. D7. A8. D9. D10.C11.C12.D13.B14.C15.D16.D17.C18.B19.D20.B21.A22.D23.C24.D25.B。

2017 AMC 8 考题及答案

美国数学竞赛AMC题目及答案

AMC考题和答案解析