2013年11月份规模以上工业增加值增长10.0%

合集下载

非物质文化遗产研究视野下的艺术人类学理论

㊀㊀㊀Ң艺术人类学(学术主持人:安丽哲)学术主持人语:本期艺术人类学栏目包含４篇文章ꎬ代表着今年的艺术人类学研究的两个重要方向ꎮ周逸煊㊁金兑玩的«民族造型艺术对现代设计的文化认同的构建»ꎬ杨娜的« 汉服的概念内涵与汉服体系的建构路径»与荣树云的«魅惑的技术:人类学视域中民间木版年画的艺术性分析»都是关于艺术符号与社会文化认同之间的关系与应用的探讨ꎬ充分体现了艺术在当今社会发展建构中的重要作用ꎬ其中«民族造型艺术对现代设计的文化认同的构建»是从宏观角度对该问题进行的探讨ꎬ后二者则是从个案角度进行的探讨ꎬ尽管研究对象不尽相同ꎬ然而大家探讨的问题却是一致的ꎬ这为我们构建本土艺术人类学的一般理论提供了参考ꎮ汪欣的«非物质文化遗产研究视野下的艺术人类学理论»则从学理角度探讨了非物质文化遗产保护与艺术人类学研究之间天然的关系ꎬ体现了艺术人类学作为交叉学科的应用性与社会价值ꎮ收稿日期:２０２０－０８－１０作者简介:汪㊀欣ꎬ中国艺术研究院艺术学研究所副研究员ꎬ研究方向:艺术人类学与非物质文化遗产保护ꎮ非物质文化遗产研究视野下的艺术人类学理论汪㊀欣(中国艺术研究院艺术学研究所ꎬ北京㊀１０００２９)摘㊀要:非物质文化遗产研究是一门应用型学科ꎬ发展于非物质文化遗产保护实践ꎬ无先行理论指导ꎮ在研究实践中ꎬ民俗学㊁文化人类学和艺术学都为其提供了一定的理论支撑和方法论指导ꎬ但依然面临理论困境和诉求ꎮ艺术人类学是以艺术为研究对象ꎬ以人类学为研究方法的学科ꎮ其艺术学和人类学的双重视角ꎬ对于非物质文化遗产研究具有重要的方法论意义ꎮ关键词:非物质文化遗产ꎻ研究渊源ꎻ理论诉求ꎻ艺术人类学中图分类号:Ｊ０－０５㊀㊀文献标识码:Ａ㊀㊀文章编号:１６７１－４４４Ｘ(２０２０)０６－００６６－０６国际ＤＯＩ编码:１０.１５９５８/ｊ.ｃｎｋｉ.ｇｄｘｂｙｓｂ.２０２０.０６.０１０ＯｎＴｈｅｏｒｉｅｓｏｆＡｒｔＡｎｔｈｒｏｐｏｌｏｇｙｉｎＶｉｅｗｏｆｔｈｅＲｅｓｅａｒｃｈｏｎＩｎｔａｎｇｉｂｌｅＣｕｌｔｕｒａｌＨｅｒｉｔａｇｅＷＡＮＧＸｉｎ(ＲｅｓｅａｒｃｈＩｎｓｔｉｔｕｔｅｏｆＡｒｔｓꎬＣｈｉｎｅｓｅＮａｔｉｏｎａｌＡｃａｄｅｍｙｏｆＡｒｔｓꎬＢｅｉｊｉｎｇ１０００２９)Ａｂｓｔｒａｃｔ:Ｔｈｅｒｅｓｅａｒｃｈｏｆｉｎｔａｎｇｉｂｌｅｃｕｌｔｕｒａｌｈｅｒｉｔａｇｅｉｓａｎａｐｐｌｉｅｄｄｉｓｃｉｐｌｉｎｅꎬｗｈｉｃｈｄｅｖｅｌｏｐｓｉｎｔｈｅｐｒａｃｔｉｃｅｏｆｉｎｔａｎｇｉｂｌｅｃｕｌｔｕｒａｌｈｅｒｉｔａｇｅｐｒｏ￣ｔｅｃｔｉｏｎｗｉｔｈｏｕｔａｎｙｐｒｉｏｒｔｈｅｏｒｅｔｉｃａｌｇｕｉｄａｎｃｅ.Ｉｎｔｈｅｒｅｓｅａｒｃｈｐｒａｃｔｉｃｅꎬｆｏｌｋｌｏｒｅꎬｃｕｌｔｕｒａｌａｎｔｈｒｏｐｏｌｏｇｙａｎｄａｒｔｓｈａｖｅｐｒｏｖｉｄｅｄｃｅｒｔａｉｎｔｈｅｏｒｅｔｉｃａｌｓｕｐｐｏｒｔｓａｎｄｍｅｔｈｏｄｏｌｏｇｉｃａｌｇｕｉｄａｎｃｅꎬｂｕｔｔｈｅｒｅｓｔｉｌｌｆａｃｅｓｔｈｅｏｒｅｔｉｃａｌｄｉｌｅｍｍａｓａｎｄａｐｐｅａｌｓ.Ａｒｔａｎｔｈｒｏｐｏｌｏｇｙｉｓａｄｉｓｃｉｐｌｉｎｅｔｈａｔｔａｋｅｓａｒｔａｓｉｔｓｒｅｓｅａｒｃｈｏｂｊｅｃｔａｎｄａｎｔｈｒｏｐｏｌｏｇｙａｓｉｔｓｒｅｓｅａｒｃｈｍｅｔｈｏｄｗｈｏｓｅｄｕａｌｐｅｒｓｐｅｃｔｉｖｅｓｏｆａｒｔａｎｄａｎｔｈｒｏｐｏｌｏｇｙｈａｖｅｉｍｐｏｒｔａｎｔｍｅｔｈｏｄｏｌｏｇｉｃａｌｓｉｇｎｉｆｉ￣６６０贵州大学学报艺术版㊀第３４卷㊀总第１２０期㊀２０２０年第６期㊀㊀㊀ｃａｎｃｅｆｏｒｔｈｅｓｔｕｄｙｏｆｉｎｔａｎｇｉｂｌｅｃｕｌｔｕｒａｌｈｅｒｉｔａｇｅ.Ｋｅｙｗｏｒｄｓ:ｉｎｔａｎｇｉｂｌｅｃｕｌｔｕｒａｌｈｅｒｉｔａｇｅꎻｒｅｓｅａｒｃｈｏｒｉｇｉｎꎻｔｈｅｏｒｅｔｉｃａｌａｐｐｅａｌꎻａｒｔａｎｔｈｒｏｐｏｌｏｇｙ㊀㊀一、非物质文化遗产研究的研究渊源与理论诉求㊀㊀(一)非物质文化遗产保护的兴起与研究渊源非物质文化遗产保护是我国在２１世纪开展的一项重要的文化发展工程ꎬ是中华民族伟大复兴计划的组成部分ꎮ自２００３年开展中国民族民间文化保护工程以来ꎬ 非物质文化遗产概念逐渐进入中国文化话语体系ꎬ用一种新的方式解读中国民族民间文化ꎮ在十多年的非物质文化遗产保护实践中ꎬ我国逐渐建立起了较为完善的保护传承机制ꎮ与此同时ꎬ有关非物质文化遗产的研究也渐次展开ꎮ作为一个来源于实践㊁无先行经验可依循的全新学科ꎬ非物质文化遗产研究主要着眼于现实保护实践ꎮ有关非物质文化遗产的研究主要有非遗保护理论研究和非遗保护实践研究ꎮ在非遗保护理论研究方面ꎬ主要涉及非遗的概念解析㊁保护原则㊁文化生态等基础理论ꎮ概念解析是非物质文化遗产研究早期的重要话题ꎮ 非物质文化遗产作为舶来词汇ꎬ在中文语境中较为陌生ꎬ对其进行本土化解读十分必要ꎮ在当时的探讨中ꎬ各领域学者从定义㊁物质与非物质遗产的关系以及非遗的分类等角度进行了分析ꎮ通过这些研究ꎬ 非物质文化遗产在中国语境中的内涵得到了较为清晰的阐释ꎬ即包括民族文化㊁民间文化以及古代宫廷文化等文化形态在内的传统文化ꎮ对非遗保护原则的研究主要着眼于非遗的原真性㊁活态流变性和整体性等原则ꎮ原真性是文化遗产保护的普遍原则ꎬ以确保文化遗产是真实的而未被歪曲ꎮ针对当前国家各级非遗名录中出现的真假混杂现象ꎬ以及各级名录项目退出机制的实施ꎬ对各类非遗项目进行辨伪十分必要ꎬ因而对非遗原真性的探索也应运而生ꎮ活态流变性是非物质文化遗产的独特属性ꎮ关于非物质文化遗产变与不变的探讨由来已久ꎬ其实质是对非遗保护与发展的辨析ꎮ非遗保护实践中常见的问题是在发展的名义下过度开发ꎬ过度利用造成了非遗原真性的丧失ꎮ非遗保护的过程是实施控制性的保护 ꎬ还是允许其发展以适应现代社会ꎬ成为争议的焦点ꎮ活态流变性的属性ꎬ决定了非遗的核心价值在于其活态存续于当下ꎬ并随着时代变迁有规律地良性发展ꎮ因此ꎬ在保护实践中ꎬ应避免因噎废食式的回避发展 ꎬ而应因时因地制宜地顺势利导ꎬ使其在当代社会获得生存空间ꎮ整体性是非物质文化遗产保护的重要原则ꎬ也是最难落到实处的原则ꎮ无论物质遗产还是非物质遗产ꎬ都不是孤立的个体ꎬ而是与其所处环境相互依存ꎮ针对当前各地以项目为主导的保护措施ꎬ提出整体性保护是为了避免文化遗产保护的碎片化ꎬ使其脱离原生土壤ꎬ成为名存实亡的文化标本ꎮ在提出整体性保护原则的基础上ꎬ研究者还就实施整体性保护的具体策略提出了设计思路ꎬ以期改变其在实践中无法落到实处的困境ꎮ整体性原则衍生出的文化生态理论是研究者关注的另一个理论热点ꎮ从文化生态失衡的文化现状的提出ꎬ到系统解读文化生态学理论ꎬ为非物质文化遗产的文化生态保护以及文化生态保护区㊁生态博物馆㊁民族文化生态村㊁传统村落等文化遗产聚集地的区域性保护提供了理论支撑ꎮ随着非遗保护事业的国际化ꎬ非遗的社区保护也受到研究者的关注ꎮ社区是非遗项目生存发展的基层环境ꎬ具有良好的群众基础和较为完整的文化生态环境ꎮ社区保护则是实现非遗在地保护和文化生态整体保护最具可操作性的保护模式ꎮ有关非遗保护实践的研究主要侧重于保护方式㊁传承教育㊁宣传推广等实际问题的研究ꎮ在保护方式的研究方面ꎬ主要围绕抢救性保护㊁生产性保护和整体性保护三种基本方式进行ꎮ抢救性保护的研究主要针对非遗项目的调查采集㊁档案和数据库建设等措施ꎬ以及由此衍生的博物馆㊁展示馆㊁传习所建设ꎮ其中ꎬ数字化保护是目前最亟需研究的现实问题ꎬ研究者主要从非遗资源数字化存储与传播㊁数字化平台的搭建以及大数据技术对非遗资源与保护中出现的问题的分析等方面ꎬ研究非物质文化遗产的数字化保护问题ꎮ生产性保护的研究主要针对其原则㊁方法㊁风险㊁政策支持等实际问题ꎮ这种保护方式的提出为合理利用方针找到了实践途径ꎬ由７６０㊀㊀㊀非物质文化遗产研究视野下的艺术人类学理论汪㊀欣此衍生出的研究主题有非遗的产业化问题以及当前文旅融合背景下ꎬ非遗项目参与文化产业㊁旅游产业的发展现状与前景探索ꎮ对整体性保护实践的研究主要是探讨文化生态保护区的建设理念㊁原则㊁途径等现实问题ꎮ对非遗传承教育的研究ꎬ除了探讨传承过程㊁传承模式㊁传承人保护等基本问题ꎬ还注重对现代社会传承人培养模式与校园教育㊁新一代传承人培训㊁非遗进校园和乡土教材以及研培计划等现实问题进行探析ꎮ对非遗宣传推广的研究主要针对非遗在当下新媒体背景下的传承特征㊁困境㊁策略等问题ꎬ比如当前的直播平台对于非遗传播模式的改变及其利弊ꎮ除了上述综合性研究ꎬ个案研究是当前非物质文化遗产的主要研究趋势ꎮ非遗个案研究以田野调查资料为依据ꎬ考察该项目的发生㊁发展过程及其与周边环境之间的关系ꎮ非遗个案研究最多的是艺术类项目ꎬ其关注点从门类艺术学中对于项目艺术本体特征研究扩展到对传承人及环境整体的研究ꎬ从注重艺术文本研究拓展到从田野作业中记录㊁挖掘艺术实践的价值ꎮ总之ꎬ非物质文化遗产研究是随着其保护实践的不断发展而逐渐深入的ꎬ是在实践中发展而来的实践性学科ꎮ无先行理论指导ꎬ以实践为依据ꎬ以专题研究为主导ꎬ是当前非物质文化遗产研究的主要特征ꎮ(二)当前非物质文化遗产研究的理论困境与诉求如前文所述ꎬ非物质文化遗产研究是以保护实践为基础发展而来的实践性学科ꎬ无先行理论指导ꎬ以专题研究代理论研究ꎮ在十多年的研究探索中ꎬ研究者从不同的研究领域逐渐整理出较为系统的理论体系ꎮ在当前的研究实践中ꎬ民俗学㊁文化人类学和艺术学都为非物质文化遗产研究者提供了一定的理论视角ꎮ民俗学方法是当前非物质文化遗产研究的重要方法ꎮ民俗事象研究和民俗整体研究是民俗学方法的两种研究取向ꎮ[１] 民俗事象研究注重对已有民俗资料的历史文献研究ꎬ民俗整体研究注重民俗事象与民众生活的内在关联性的研究ꎮ [１]两种研究取向在非遗研究中都得到普遍运用ꎮ一方面ꎬ研究者用民俗事象研究的方式对非遗项目进行历史考察和文献整理ꎬ为非遗资源普查和项目保护措施的制定提供了理论依据ꎻ另一方面ꎬ研究者用民俗整体研究的方式ꎬ综合考察非遗项目与当地环境之间的互动性ꎬ为非遗的文化生态保护提供了依据ꎮ其中ꎬ民俗学的社区研究 [２]和村落调查 [３]为非遗的区域性保护提供了研究范式ꎮ在文化生态保护区建设和传统村落保护的研究中ꎬ 社区研究和村落调查为其提供了理论和方法论指导ꎮ文化人类学是一门以社会文化为研究对象的应用性社会科学ꎬ也是非物质文化遗产研究的另一个重要理论渊源ꎮ在文化人类学介入之前的文化遗产保护ꎬ主要关注其物质属性ꎬ所涉及的领域主要为考古学和文物修复ꎮ随着文化人类学的介入ꎬ文化遗产的文化属性开始受到关注ꎬ人们开始研究与之相关的文化表现形式ꎬ如传统㊁仪式㊁社会实践㊁传承模式等ꎮ在非遗研究实践中ꎬ文化人类学提供了以下理论指引ꎮ首先ꎬ尊重不同文化的独立性和价值对等性ꎬ倡导世界文化多样性发展ꎮ这一理念成为国际社会倡导世界文化多样性㊁尊重民族文化独立发展的价值基础ꎮ研究者在研究不同区域和民族文化的时候ꎬ要尊重当地民众的文化主体地位和本土文化的独特价值ꎬ不盲目以外来者视角判断其价值高低ꎮ其次ꎬ整体性是文化人类学的核心概念之一ꎬ也是其基本立场和观察方法ꎮ该理念强调从共时性和历时性双重角度考察人类社会文化ꎬ注重社会局部要素与整体的有机结合ꎮ非遗的整体性原则正是缘于文化人类学的整体论方法ꎮ在保护非物质文化遗产的时候ꎬ除了考察其在当代社会的存续状态ꎬ还要纵向全面考察其历史发展脉络和传承谱系ꎬ尊重其活态流变性ꎬ防止固化保护ꎬ使其停留在某个历史节点ꎬ而是促进其在当代社会的生存和发展ꎮ最后ꎬ进化论学派提出的文化生态学理论为非遗的文化生态保护提供了直接的理论指导ꎮ文化生态学对文化要素与环境之间互动关系的阐释ꎬ解释了非遗项目与所处环境之间的关系ꎬ有助于更好地实现区域整体保护ꎮ民俗学和文化人类学都从文化视角解读非物质文化遗产ꎬ却都忽略了艺术类非遗项目的艺术属性ꎮ要系统全面地研究非物质文化遗产ꎬ仅仅观照其文化属性ꎬ从文化学角度对其进行解析是不够的ꎬ还应有艺术学科的介入ꎮ艺术类非遗项目在我国非遗名录中占有较高比重ꎬ如表演艺术类的传统音乐㊁舞蹈㊁戏剧㊁曲艺以及竞技与杂技ꎬ造型艺术类的传统美术与８６０贵州大学学报艺术版㊀第３４卷㊀总第１２０期㊀２０２０年第６期㊀㊀㊀手工技艺ꎮ因此ꎬ对于艺术类非遗项目ꎬ艺术学是不可或缺的研究方法ꎮ艺术学是以整个艺术为研究对象的学科ꎮ其研究方式ꎬ除了传统的思辨研究和艺术本体研究ꎬ还将文化学研究方法引入其中ꎬ 把艺术世界放在它的一个最切近的上位系统即文化的系统ꎬ也就是精神生活㊁精神文明㊁精神生产的语境来观照㊁透视艺术的种种规律性现象ꎬ探讨艺术在一定时代㊁一定民族㊁一定社会环境下的精神创造活动中的地位及意义 [４]１３４ꎮ这种艺术学上的整体研究ꎬ注重艺术语境 [４]１４８的研究ꎬ从整体上思考艺术与语境的关系ꎮ此处的语境同民俗学㊁文化人类学的环境相同ꎬ指文化要素所处的社会文化环境ꎮ综上所述ꎬ民俗学㊁文化人类学和艺术学都为非物质文化遗产研究提供了一定的理论和方法论支撑ꎬ是其研究的重要理论渊源ꎮ然而ꎬ非物质文化遗产只是作为它们的一个研究点或者说研究方向ꎬ非物质文化遗产作为一门学科没有形成独立的理论与方法论体系ꎮ这三种基础学科从各自的理论视角解读非物质文化遗产ꎬ为我们展示了非物质文化遗产不同的解剖面ꎬ但无法完整系统地构建起其独立的研究体系ꎮ非物质文化遗产研究亟需一种能够全面阐释其文化㊁艺术㊁社会等各方面价值的新的理论视野ꎮ㊀㊀二、艺术人类学理论与研究实践(一)艺术人类学的理论与方法论特征艺术人类学是艺术学和人类学的交叉学科ꎬ融合了艺术学和人类学的双重研究特征ꎬ既是一门有关艺术的理论ꎬ也是一种实践性的研究方法ꎮ 其研究对象㊁内容和目的是艺术学的ꎬ研究视角和方法是人类学的ꎮ [５]艺术人类学的研究对象与内容是艺术ꎮ首先ꎬ从关注艺术品到关注艺术行为ꎮ艺术人类学更加重视研究艺术行为ꎬ认为作品是艺术行为的组成部分ꎬ要将作品置于艺术生产㊁流通和接受的社会语境之中进行研究ꎮ在对艺术行为过程的研究中ꎬ尤其注重对艺术家群体的研究ꎬ认为艺术家是艺术行为的核心ꎮ因此ꎬ参与艺术行为的人是艺术人类学研究的重要内容之一ꎮ在研究艺术行为人的过程中ꎬ除了关注其个体化的艺术创作ꎬ还关注其生存状态㊁社会环境及其他影响到艺术创作行为的活动ꎮ其次ꎬ从关注艺术本体到关注艺术语境ꎮ艺术人类学除了关注艺术本体ꎬ更注重将艺术本体置于特定的社会文化语境之中ꎬ研究艺术本体的内涵㊁意义及价值ꎮ第三ꎬ从关注传统艺术到关注现代文明社会中的艺术ꎮ早期的艺术人类学主要关注原始艺术㊁土著艺术和民间艺术ꎬ当代艺术人类学的研究领域逐渐拓展到现代社会中的艺术ꎮ当前的艺术人类学ꎬ不仅研究传统艺术及其生存发展的文化语境ꎬ更加注重研究传统艺术在现代商业化社会环境中的适应与变迁ꎮ最后ꎬ从关注异域文化中的艺术到关注本土艺术ꎮ早期的艺术人类学注重研究异文化中的艺术ꎬ当代艺术人类学则更加注重对本土艺术的研究ꎮ艺术人类学的研究视角和研究方法都是人类学的ꎮ首先ꎬ以文化整体观为理论基础ꎬ强调艺术与社会文化机构之间的关系是局部与整体的关系ꎬ注重研究二者之间的联系ꎬ既关注艺术本体ꎬ还关注艺术本体所产生的社会结构与文化体系ꎬ探讨艺术本体的文化内涵ꎮ其次ꎬ对不同区域和族群的艺术进行跨文化比较研究ꎬ强调各社区文化的主体性ꎬ尊重社区主体对自身艺术或审美活动的解说ꎬ尊重研究对象及其文化的主体地位ꎮ第三ꎬ以田野工作和艺术民族志为学科标志ꎮ人类学田野注重对异域文化的考察ꎬ艺术人类学则更为关注本土文化ꎬ尤其重视研究少数民族地区㊁偏远山村地区以及城市边缘地区的艺术形式和艺术群体ꎮ最后ꎬ跨学科方法的运用是艺术人类学的又一特征ꎬ除了艺术学和人类学ꎬ历史学㊁社会学㊁考古学等学科也为其提供了方法借鉴ꎮ与人类学对艺术的研究不同ꎬ艺术人类学研究的最终学术目标是艺术ꎬ是置于文化之中的艺术ꎮ艺术人类学在研究艺术本体的同时ꎬ注重了解其背后的社会文化制度ꎮ这种双重视角可以消除艺术研究与文化研究之间的分歧ꎮ(二)艺术人类学的研究实践２００６年１２月ꎬ中国艺术人类学会在北京成立ꎬ标志着艺术人类学在中国的本土化ꎮ此后ꎬ中国学者对艺术人类学的研究不断深入㊁拓展ꎬ将艺术人类学从理论带入了实践ꎬ从审美研究带入了田野现场ꎮ艺术人类学的研究不断与中国社会发展紧密结合ꎬ将研究视角置于与社会发展息息相关的文化和艺术实践中ꎮ艺术人类学第一个与中国社会发展紧密结合的研究实践是将非物质文化遗产纳入研究视野ꎮ２００７年６月初ꎬ中国艺术研究院与台湾东吴大学在北京共同举办了一次非物质文化遗产保护中的田野考察工９６０㊀㊀㊀非物质文化遗产研究视野下的艺术人类学理论汪㊀欣作方法研讨会 [６]ꎬ这不仅是中国艺术人类学会成立之后承办的第一次研讨会ꎬ会议以非物质文化遗产为主题ꎬ将田野考察作为艺术人类学的基本研究方法ꎮ此后ꎬ非物质文化遗产成为艺术人类学重要的研究主题ꎮ在历届研讨会提交的论文中ꎬ有关非物质文化遗产的研究主要涉及非遗保护理论和非遗项目个案调查两个方面专题ꎮ有关非遗保护理论的研究ꎬ主要探讨非遗保护中的基本概念解读㊁物质与非物质文化之辨㊁保护与传承主体㊁保护方式与传承模式等保护实践中出现的现实问题ꎮ非遗项目个案调查主要是运用艺术人类学的方法对具体项目进行全方位的综合性考察与分析ꎮ艺术人类学另一个主要研究对象是门类艺术ꎬ包含了表演艺术和造型艺术ꎮ艺术人类学视角中门类艺术研究的特点是:首先ꎬ从艺术本体出发ꎬ记录㊁挖掘其完整的艺术过程ꎻ其次ꎬ通过历史文献研究ꎬ挖掘其历史渊源和文化内涵ꎻ第三ꎬ考察其生存㊁发展的社会文化环境ꎬ将艺术本体置于环境土壤中进行整体研究ꎻ最后ꎬ将田野调查和民族志研究作为基本方法论ꎮ非物质文化遗产中艺术类项目的个案研究ꎬ除了具有门类艺术研究的基本特点ꎬ还侧重在此研究基础上提出相应的保护和传承措施ꎮ随着艺术研究者参与非物质文化遗产保护实践ꎬ他们的研究也逐渐倾向于解决艺术项目保护与传承实践中的现实问题ꎮ此外ꎬ与艺术和非物质文化遗产都紧密关联的民俗文化也是艺术人类学研究的一个重要方面ꎮ民俗是一个地区最具综合性的文化现象ꎬ函括了当地多种文化和艺术形式ꎮ这些文化艺术形式不仅构成当地民俗文化的组成要素ꎬ还以民俗文化为生存环境ꎬ在民俗活动中获得生存空间ꎮ因此ꎬ研究当地的文化艺术ꎬ不能忽视当地的民俗文化ꎮ近年来ꎬ随着国家乡村振兴战略的提出和新一轮乡村建设浪潮的兴起ꎬ艺术乡村建设成为艺术界和乡建领域共同参与的话题ꎮ艺术人类学将艺术乡村建设研究作为新的研究主题ꎬ这也是艺术人类学第二次与中国社会发展紧密结合的研究实践ꎮ艺术乡村建设不是单一的乡村建设活动ꎬ而是融合了复兴乡村社会传统文化ꎬ以艺术改造传统村落的艺术实践活动ꎮ艺术家的介入为乡村建设注入了活力ꎬ然而纯粹的艺术化改造必然造成村落的艺术标本化ꎮ艺术人类学从艺术和文化的双重理论视角ꎬ为艺术乡村的建设提供理论支撑和实践指导ꎬ使村落充满艺术色彩而不失精神文化内核ꎮ㊀㊀三、艺术人类学对非物质文化遗产研究的方法论意义㊀㊀如前文所述ꎬ非物质文化遗产研究是一门应用型学科ꎬ无先行理论指导ꎬ是在实践中不断总结探索出研究经验ꎬ以专题研究的形式来解决现实中遇到的问题ꎮ综合前文所述的民俗学㊁文化人类学和艺术学理论与方法在非遗研究中的运用ꎬ笔者认为ꎬ艺术人类学更能为非物质文化遗产研究提供理论支撑和方法论指导ꎮ艺术人类学与非遗研究在研究对象上具有一致性ꎮ艺术人类学以艺术为研究对象ꎬ研究内容涉及民间艺术㊁非物质文化遗产以及民俗文化ꎮ非遗研究以非物质文化遗产为研究对象ꎬ研究内容包含了表演艺术㊁造型艺术以及民俗文化在内的所有非物质文化遗产ꎮ非遗中的艺术不是纯粹的审美艺术ꎬ而是具有本土文化色彩的生活化的艺术ꎮ对这种艺术形式的研究ꎬ不仅要有艺术视角ꎬ还要有文化学视角ꎮ艺术人类学的双重视角能为其提供更为系统全面的理论支撑ꎮ艺术人类学研究以文化整体观为基本学术立场ꎬ对艺术进行整体性研究ꎬ不仅研究艺术本体ꎬ还将其置于社会文化环境中进行系统考察ꎮ整体性也是非遗保护的基本原则ꎮ在这一原则下ꎬ非遗保护不再只是对单一项目进行保护ꎬ而是将该项目置于其存续的文化生态环境ꎬ重视文化生态的维护和修复ꎮ在研究非物质文化遗产单一项目时ꎬ也将其视为一个小的环境系统或者行为过程 ꎬ采取整体性视角进行解读ꎮ然而ꎬ非遗保护的整体性原则并没有落实到具体保护实践中ꎬ更多地停留在空洞条文而无具体措施ꎮ艺术人类学不仅能从理论上为非遗保护提供整体性研究的体系框架ꎬ还能在实践中指导其将整体性保护原则落实到具体措施之中ꎮ艺术人类学与非遗研究都注重对人的研究ꎮ艺术人类学注重考察艺术家及其群体的生存状态ꎬ以获取第一手民族志资料ꎬ了解研究对象与社会文化的关系ꎮ在田野调查过程中ꎬ 艺术人类学者通常采用口述史的方式ꎬ记录艺术家及艺术群体对研究对象的发生㊁发展历程的描述ꎬ以全面㊁真实地展现研究对象的全貌ꎮ [７]非遗研究以传承人为非物质文化遗产传承发展的核心要素ꎮ在研究过程中ꎬ通过考察传０７０。

Proof of Riemann's zeta-hypothesis

Proof of Riemann’s zeta-hypothesisArne BergstromB&E Scientific Ltd, Seaford, BN25 4PA, United KingdomSubmitted 27 July 2008; revised 27 September 2008______________________________________________________________________________________ AbstractMake an exponential transformation in the integral formulation of Riemann's zeta-function ζ(s)for Re(s)> 0. Separately, in addition make the substitution s −>1-s and then transform back to s again using the functional equation. Using residue calculus, we can in this way get two alternative, equivalent series expansions for ζ(s) oforder N, both valid inside the "critical strip", i e for 0<Re(s)<1. Together, these two expansions embody important characteristics of the zeta-function in this range, and their detailed behavior as N tends to infinitycan be used to prove Riemann's zeta-hypothesis that the nontrivial zeros of the zeta-function must all have real part ½.Keywords: Riemann’s zeta-function; Critical strip; Nontrivial zeros; Functional equation; Residue calculus______________________________________________________________________________________ 1. IntroductionRiemann's zeta-hypothesis from 1859 [9] is expressed as follows,Conjecture 1.1.The nontrivial zeros of the Riemann zeta-function ζ(s) all have real part Re(s)= ½.The Riemann zeta-hypothesis is the most famous of the few still unsolved problems on Hilbert's list of twenty-three mathematical challenges, which he presented in 1900 at the dawn of the new century [10, 16]. It is also one of the seven Millennium Problems [17] named in 2000 by the Clay Mathematics Institute.It can be shown (cf [13]) that the nontrivial zeros of the zeta-function must lie inside the "critical strip", i e for0 < Re(s)< 1, which is the range studied in this paper.The Riemann zeta-hypothesis has been computationally verified for |Im(s)| at least up to 2.4 trillion [15].The intriguing possibility has been suggested that the Riemann zeta-function could correspond to a quantum-physical problem with its zeros corresponding to energy eigenvalues. The underlying physical problem would then correspond to a chaotic quantum system without time-reversal symmetry [4, 5]._______E-mail address: arne.bergstrom@With (σ and t are real)= s + σi tRiemann's zeta-function ζ(s ) can be defined as the following series, convergent for σ > 1,= ()ζs ∑ = n 1∞1n sThis Dirichlet series can also be expressed as follows (for σ > 1),= () − 12()−s ()ζs ∑ = n 1∞1() − 2n 1sIn Sects 2 through 5 below a modification of this latter series will be derived, giving the equivalent pair (9) and (11), which are valid also inside the critical strip. Although it will be shown that (9) and/or (11) are somewhat similar to previous results found in the literature,the approach described in the following permits a more detailed analysis, leading to a proof of Conjecture 1.1.The proof of Riemann’s zeta-hypothesis given in this paper is based on the following two fundamental properties of the Riemann zeta-function:the integral representation (1), valid for Re (s ) > 0 [8, 12],= () − 12() − 1s ()Γs ()ζs d ⌠⌡⎮⎮⎮⎮⎮0∞w () − s 1 + e w 1w(1)the functional equation (2), valid for all s [6, 11],= ()ζs 2s π() − s 1⎛⎝⎜⎜⎞⎠⎟⎟sin 12s π()Γ − 1s ()ζ − 1s(2)2. Variable transformationWe start by transforming the variable w in (1) as follows= w e ui e= () − 12() − 1s ()Γs ()ζs d ⌠⌡⎮⎮⎮⎮⎮⎮⎮−∞∞()e u s + e ()e u 1u(3)The integration variable w in (1) being real, we can also set u real. Then= () − 12() − 1s ()Γs ()ζs d ⌠⌡⎮⎮⎮⎮⎮⎮−∞∞e ()s u + e ()e u 1u(4) Consider the integrand=()F u e ()s u + e ()e u 1 (5)and extend u to the entire complex plane,= u + x i yExtended over the complex plane, F (u ) is an analytic (meromorphic) function .3. Poles and residuesWe next calculate the poles of F (u ) above, i e we want to find all u that satisfy the equation= + e ()e u 10which can be verified to have the following solutions (m and n are integers, n > 1),= u + ()ln π() − 2n 1i π⎛⎝⎜⎜⎞⎠⎟⎟ + 12m The poles are thus all situated in the half-plane x > 0, and are symmetric around the real axis in conjugate pairs at half-integer values of π in the positive and negative imaginary directions. The residues of F (u ) corresponding to these poles are given by the following expression= ()Res ,n m i ()-1m () − 2n 1() − s 1π() − s 1e ()i () + /12m s π Let S N be the sum of the residues in the strip 0 < y < 2 π (i e for m = 0 and m = 1), and from n = 1 up to and including the pair of residues at x = ln((2N-1) π). Then= S N 2⎛⎝⎜⎜⎞⎠⎟⎟sin 12s πe ()i s ππ() − s 1⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N() − 2n 1() − s 1 (6) (6), as well as (7) below, both tend to infinity with N for 0 < Re (s ) < 1. Note however Remark 5.2 in Sect 5 below.4. Contour integralConsider now a closed contour C N in the complex plane consisting of the real axis in the positive direction from x = − e to x = L just to the right of the pair of residues mentioned above at x = ln((2N-1) π), then a vertical connection from y = 0 to y = 2π at x = L up to a line from x = L back to x = − e in the negative direction parallel to the real axis and at a distance 2π above it, and then finally a vertical connection at negative infinity back down from y = 2π to y = 0. This contour encloses the N pairs of residues summed as S N in (6)above, and is here traversed in the positive direction.Theorem 4.1. The integral I N of (5) around the contour C N as defined above is= I N − i 2() + s 1N s πs e ()i s π⎛⎝⎜⎜⎞⎠⎟⎟sin 12s π()ΕN s 2i e ()i s π()sin s π() − 12() − 1s ()Γs ()ζs (7)where ΕN (s ) is an error factor incorporating truncation errors.Proof. See Appendix A.5. Two equivalent expressions for ζ(s )Now use Cauchy's theorem to equate the contour integral I N in (7) to the sum of residues S N in (6),= I N 2i πS Ni e − i 2() + s 1N s πs e ()i s π⎛⎝⎜⎜⎞⎠⎟⎟sin 12s π()ΕN s s 2i e ()i s π()sin s π() − 12() − 1s ()Γs ()ζs =4i ⎛⎝⎜⎜⎞⎠⎟⎟sin 1s ππs ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1() − s 1e ()i s π (8)Solve for ζ(s ),= ()ζs πs ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− + 2() − s 1N s ()ΕN s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1() − s 1s ⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 12() − 1s ()Γ + s 1 (9)An equivalent expression can be obtained by making the substitution s -> 1- s in (9),= ()ζ − 1s π() − 1s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− + 2()−s N () − 1s ()ΕN − 1s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1()−s () − 1s ⎛⎝⎜⎜⎞⎠⎟⎟sin s π()− + 12s ()Γ − 2s (10)and then transforming back to ζ(s ) again by using the functional equation (2),= ()ζs − + N() − 1s ()ΕN − 1s 2s⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1()−s () − 1s ()− + 12s () − 1s (11)From (A9) in Appendix A, the error factors in (9) and (11) can be written as= ()ΕN s + + 1s () − s 1()ενs N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3(12a) = ()ΕN − 1s + + 1s () − s 1()εν − 1s N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3 (12b)Remark 5.1. The two equivalent expressions (9) and (11) above are somewhat analogous to the two equivalent expressions obtained by an integral and the same integral integrated by parts. In fact, performing the analogous operations as above on, e g, Euler's integral form [1]of the related gamma-function [ in that case the substitution s −> s + 1 followed by the functional equation Γ(s ) = Γ( s + 1)/s ] yields precisely the same result as integrating by parts.Remark 5.2. It should be emphasized that (8), from which (9) and (11) were derived, is Cauchy’s theorem, which thus rigorously connects the power N s in the first term to the zeta-function in the second term and to the sum over N on the right-hand side. Since all functions involved are analytic also in the limit N −> e , this exact relationship between the terms is thus maintained to give finite results for ζ(s ) also in the limit N −> e , even though the two contributions in (8) from (6) and (7) are then both divergent.Remark 5.3. It is interesting to compare (11) above with the Dirichlet series valid for σ > 1mentioned in the Introduction. Insert (12b) from above, and (A8) from Appendix A into(11),= () − 12()−s ()ζs − + N () − 1s ⎛⎝⎜⎜⎞⎠⎟⎟ − + 1124s () − s 1N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 32s () − 1s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N 1() − 2n 1s Comparing this with the Dirichlet series in the Introduction, we see that the last term on the right-hand side above is a finite form of the Dirichlet series. However, in contrast to that series, which is divergent for σ < 1, the present relationship for ζ(s ) is derived from (1) and is thus valid also for s in the critical strip, i e also for 0 < σ < 1. This is a result of the rigorous derivation of (11) from Cauchy’s theorem, and is effected by the first term on the right-hand side above tracking the behavior of the Dirichlet series as N tends to infinity in order to give a correct rendering of the zeta-function.Remark 5.4. By using alternative ways to extend the integrand in (3) to an analytic function on the complex plane, it is possible by the same technique as above to obtain variants of (9)and (11) [e g, by variations on the step from (3) to (4)]. Also other approaches lead to similar (but not identical) expressions for ζ(s ), e g, the sum of the first N terms of its Dirichlet series plus a power in N as in (11) [14]. The particular variants (9) and (11) above are selected here since their properties turn out to be fortuitously well suited for the following proof of Conjecture 1.1, the Riemann zeta-hypothesis.6. Proof of Conjecture 1.1The two equivalent expressions for ζ(s ) in (9) and (11) above should be understood as follows. For each N there exists a particular function ΕN (s ) in (12a) within its Landau O(1/N 3 ) such that (9) is exactly true. Thus for the right-hand side of (9) with this particular ΕN (s ), the functional equation is exactly true also after the substitution in (10). This thus means that there exists a particular function ΕN (1 - s ) in (12b) within its O(1/N 3 ) such that also (11) holds exactly.Now consider the following two functions in the range 0 < σ < 1,= ()ζN s 'πs⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− + 2() − s 1N s ()ΕN s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1() − s 1s ⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 12() − 1s ()Γ + s 1 (13) = ()ζN s ''− + N() − 1s ()ΕN − 1s 2s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1()−s () − 1s ()− + 12s () − 1s (14)where the notation ζN (s ) is here used to mark truncation at n = N in the series expansions,and where prime and double-prime, respectively, are used to separate the two forms. Note that (13) and (14) of course are identical to (9) and (11) for the particular functions ΕN (s ) and ΕN (1 - s ) just discussed.However, in (13) and (14) we let the functions ζN (s )´ and ζN (s )´´ describe also all approximate functions around ζ(s ) obtained when we let the errors εν in ΕN (s ) and ΕN (1 - s )in (12a) and (12b) attain all possible (finite) values. For finite N, the functions ζN (s )´ and ζN (s )´´ are then of course normally no longer equivalent. Specifically, for finite N the following differences between the approximate functions in (13), (14) and the exact zeta-function will normally be nonzero (and unequal),= − ()ζN s '()ζs − πs ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− + 2() − s 1N s ()ΕN s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1() − s 1s ⎛⎝⎜⎜⎞⎠⎟⎟cos 1s π()− + 12() − 1s ()Γ + s 1()ζs (15) = − ()ζN s ''()ζs − − + N() − 1s ()ΕN − 1s 2s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1N () − 2n 1()−s () − 1s ()− + 12s () − 1s ()ζs (16)In Appendix B, the quotient of (15) and (16) is calculated in closed form to give = − ()ζN s '()ζs − ()ζN s ''()ζs + 1N ()− 2s 1πs ()− + 4s 8s ⎛⎝⎜⎜⎞⎠⎟⎟cos 2s π()− + 22s ()Γ − s 3() + s 2() + s 1s ()O N () − 2σ2 (17) We shall now study this quotient in the limit N −> e . As seen by writing N s as N σ exp(i t ln(N )), the argument of N s becomes indeterminate on the unit circle in the limit N −> e . Thus when limits of type N s are concerned, it is only relevant to consider their moduli, as we shall do in the following.In the limit N −> e , all error factors ΕN (s ) and ΕN (1 - s ) as given in (12a) and (12b) become equal to unity. Hence in this case too, (13) and (14) become identical to (9) and (11) as above, i e we have |ζN (s )´| = |ζN (s )´´| = |ζ(s )| for all s , including (by continuity) the case when |ζ(s )| is zero. In the limit N −> e , the quotient |ζN (s )´/ ζN (s )´´| thus becomes unity for all s.But in the limit N −> e , (15) and (16) above will then consequently also both be zero.Hence in this limit , the quotient on the left-hand side of (17),lim → N ∞− ()ζN s '()ζs − ()ζN s ''()ζs will be of type |0/0|, and is thus in general indeterminate between zero and infinity (for a fuller discussion of this point, see Remark B.3 in Appendix B).However, at least for zeros of the zeta-function, ζ(s ) = 0, the quotient above has a definite value. For in that case the quotient obviously becomes |ζN (s )´/ ζN (s )´´| for all N , and in the limit N −> e this becomes unity for all s as discussed above. At zeros of the zeta-function and in the limit N −> e , (17) thus becomes= lim → N ∞12N () − 2s 1πs () − 4s 8s ⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 22s () + s 2() + s 1s ()Γ − s 31 (18) This equation can be true only if the modulus of N ( 2 s - 1) is equal to N 0 = 1, which requiresthat = σ12This thus proves Conjecture 1.1 that Re (s ) must be equal to ½ for all zeros of the Riemann zeta-function ζ(s ) in the range 0 < Re (s ) < 1.Remark 6.1. It should be noted that also other values of ζ(s ) than ζ(s ) = 0 can make the quotient on the left-hand side in (17) become unity and give (18), and thus the above value of σ. However, among the ζ(s ) that have this property, we must with necessity find also every nontrivial zero of the zeta-function, as was shown above (cf Remark B.3 in Appendix B).Remark 6.2. Parenthetically, we note that for consistency the rest of the expression on the left-hand side of (18) should also become unity for σ = ½. Since for s = ½ + i t we have |(s + 2) (s + 1) s Γ(s − 3)| = |Γ(s )|, then the left-hand side of (18) can be calculated as follows for s = ½ + i t , where the last equality is a known property [2] of the gamma-function,12πs () − 4s 8s ⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 22s ()Γs =π()cosh πt ⎛⎝⎜⎜⎞⎠⎟⎟Γ + 12i t =1A PPENDIX A. Proof of Theorem 4.1A1. Contour integral I N . The integral I N of (5) around the closed contour C N defined in Sect 4 can be written as follows,= I N + − − d ⌠⌡⎮−∞L ()F x x i d ⌠⌡⎮02π()F + L i y y d ⌠⌡⎮−∞L ()F + x 2i πx i d ⌠⌡⎮02π()F − + ∞i y y Here the first term in I N is the (transformed) Riemann integral with finite upper limit x = L ,= d ⌠⌡⎮−∞L ()F x x d ⌠⌡⎮⎮⎮⎮⎮⎮−∞Le ()s x + e ()e x 1x The second term in I Nis the integral of (5) from y = 0 to y = 2 π for x = L , = i d ⌠⌡⎮02π()F + L i y y i d ⌠⌡⎮⎮⎮⎮⎮⎮⎮02πe ()s () + L i y + e ()e () + L i y 1y The third term in I N , i e the integral of (5) from x = L down to x −> − e along y = 2 π, can be shown to be a factor times the first term above,= −d ⌠⌡⎮−∞L ()F + x 2i πx −d ⌠⌡⎮⎮⎮⎮⎮⎮⎮−∞Le ()s () + x 2i π + e ()e () + x 2i π1x i e= −d ⌠⌡⎮−∞L ()F + x 2i πx −e ()2i s πd ⌠⌡⎮⎮⎮⎮⎮⎮−∞Le ()s x + e ()e x 1xThe fourth term in I N , i e the vertical connection from y = 2 π down to y = 0 at x −> − e ,can be shown to tend to zero (for σ > 0),= −i d ⌠⌡⎮02π()F − + ∞i y y 0 As shown in Sect 3, the integrand has poles at certain values of x and y . In the following,L is assumed to be chosen to stay clear of those poles, or specifically (N is an integer,N > 1), = L ()ln 2N π Using the above results, the integral I N can be rewritten as= I N + I 1I 2where= I 1() − 1e ()2i s πd ⌠⌡⎮⎮⎮⎮⎮⎮−∞()ln 2πN e ()s x + e ()e x 1x = I 2i d ⌠⌡⎮⎮⎮⎮⎮⎮⎮02πe ()s () + ()ln 2πN i y + e ()e () + ()ln 2πN i y 1y A2. Integral I 1 . The integral I 1 above can be rewritten as= I 1 − () − 1e ()2i s πd ⌠⌡⎮⎮⎮⎮⎮⎮−∞∞e ()s x + e ()e x 1x () − 1e ()2i s πd ⌠⌡⎮⎮⎮⎮⎮⎮()ln 2πN ∞e ()s x + e ()e x 1x Use (4) to express the first integral in terms of the Riemann zeta-function, and transform the second integrand back to its original form,= I 1 − () − 1e ()2i s π() − 12() − 1s ()Γs ()ζs () − 1e ()2i s πd ⌠⌡⎮⎮⎮⎮⎮2πN ∞w () − s 1 + e w 1w For 0 < Re (s ) < 1, the last term can be estimated as follows, < d ⌠⌡⎮⎮⎮⎮⎮2N π∞w () − s 1 + e w 1w d ⌠⌡⎮⎮⎮⎮⎮2N π∞w 0 e w wi e < d ⌠⌡⎮⎮⎮⎮⎮2N π∞w () − s 1 + e w 1w e ()−2N π Since the exponential factor in front of the last integral in I 1 is always bounded, we then have = I 1− + 2i e ()i s π()sin s π() − 12() − 1s ()Γs ()ζs ()O e ()−2πN A3. Integral I 2 . The integral I 2 in Sect A1 can be expanded as follows= I 2i 2s πs N s d ⌠⌡⎮⎮⎮⎮⎮⎮02πe ()i s y + e ()2πN () + ()cos y i ()sin y 1y(A1) (A1) has the following general behavior. When cos (y ) is positive, i e for 0 < y < π/2 and 3 π/2 < y < 2 π, then the denominator tends to infinity exponentially with N , and the integrand tends to zero. On the other hand, when cos (y ) is negative, i e for π/2 < y < 3 π/2,then the exponential term in the denominator tends to zero when N −> e , and the integral reduces to= I 2i 2s πs N s d ⌠⌡⎮⎮/12π/32πe ()i s y y (A2)which integrates to= I 2−2s πs N s ()− + e ()/32i s πe ()/12i s πs (A3)In the limit N −> e , the integrand in (A1) is thus a step function, which is zero for 0 < y < π /2 and for 3 π/2 < y < 2 π, whereas for π /2 < y < 3 π /2 the integrand is given as in (A2). However, the two special cases when cos (y ) is close to zero, i e around y = π /2 and y = 3 π /2, respectively, merit separate study. These regions will be the centers for a correction to the integrated result in (A3), which for large N can be calculated as follows. Study here first the behavior around y = π /2. After Taylor expansions around π /2 in the numerator and denominator in (A1), the integral I 2 can be written there as= ⎛⎝⎜⎜⎞⎠⎟⎟I 2,12πδi e ()/12i s π2s πs N s d ⌠⌡⎮⎮⎮⎮⎮⎮⎮⎮⎮ − /12πδ+ /12πδ + − + 1i s ⎛⎝⎜⎜⎞⎠⎟⎟ − y 12π12s 2⎛⎝⎜⎜⎞⎠⎟⎟ − y 12π2 . . . + e ()2πN () − + − + + i y /12π/12i () − y /12π2/16() − y /12π3 . . .1y where the interval δ is chosen so that it (at least) covers the region of appreciable deviation from the step function in (A2), as will be further discussed below.After changing integration variable,= N ⎛⎝⎜⎜⎞⎠⎟⎟ − y 12πτand noting that exp(2N π i ) = 1, the expression above can be rewritten as follows (truncating at powers in 1/N of order two in the integrand, collecting the remainders into the numerator)= ⎛⎝⎜⎜⎞⎠⎟⎟I 2,12πδi e ()/12i s π2s πs N s d ⌠⌡⎮⎮⎮⎮⎮⎮⎮⎮⎮⎮−N δN δ+ + 1i s τN 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3 + e ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− − 2πτi πτ2N 1τRemark A3.1. Define ∆I 2 (π/2) as the correction when we approximate the integral in (A1)around y = π/2 by the integral in (A2) over the step function there. It can be expressed as the above integral minus the integral of the corresponding step function (i e with the exponential in the denominator equal to zero, and the lower limit equal to zero), and integrated over the region around y = π/2 defined by − N δ < τ < N δ where the integrands differ appreciably, i e more than O(1/N 3 ). Since N δ can always be chosen larger than this minimum requirement,some freedom is allowed in the choice of N δ (cf Remark A3.3 below).= ∆⎛⎝⎜⎜⎞⎠⎟⎟I 212πi e ()/12i s π2s πs N s ⎛⎝⎜⎜⎜⎜⎜⎜⎜⎜⎜⎜⎜⎜⎞⎠⎟⎟⎟⎟⎟⎟⎟⎟⎟⎟⎟⎟ − d ⌠⌡⎮⎮⎮⎮⎮⎮⎮⎮⎮⎮−N δN δ + + 1N i s τN 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3 + e ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− − 2πτi πτ2N 1τd ⌠⌡⎮⎮⎮⎮0N δ + + 1N i s τN 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3τ After expanding the first integrand as a Taylor series in 1/N , we get= ∆⎛⎝⎜⎜⎞⎠⎟⎟I 212πi e ()/12i s π2s πs N s ⎛⎝⎜⎜⎜⎜⎜⎜⎜⎞⎠⎟⎟⎟⎟⎟⎟⎟ − d ⌠⌡⎮⎮⎮⎮⎮−N δN δ + + a 1N a 2N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3τd ⌠⌡⎮⎮⎮⎮0N δ + + 1N i s τN 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3τ(A4)where= a 11+ e ()−2πτ1=a 2 +i s τi e ()−2πτπτ2+ e ()−2πτ1 + e ()−2πτ1Remark A3.2. The correction ∆I 2(π/2) above corresponds to the integral of a more or less sharp peak (depending on N ) at y = π/2, i e at τ = 0. This peak is well described by the functions of τ in a 1, a 2, and in the second integrand in (A4) above. The series expansions in 1/N in (A4) are required only to second order for the following calculations.Inserting the above expressions for a 1 and a 2 into (A4), and integrating for each power of N , we get= + d ⌠⌡⎮⎮⎮⎮−N δ0a 1N τd ⌠⌡⎮⎮⎮⎮0N δ − a 1N 1N τ0 (A5) = + d ⌠⌡⎮⎮⎮⎮⎮−N δ0a 2N 2τd ⌠⌡⎮⎮⎮⎮⎮0N δ − a 2N 2i s τN 2τi () − s 1()ενs N 2 (A6)where the factor εν (s ) = ε (s , N δ ) is a function of s and the limit N δ = ν, and can be expressed in exact, explicit form using Euler’s dilogarithm function Li 2(x ) [3, 7] as follows,= ()ενs + − + 14 − ()Li 2 + e ()2νπ1()Li 2 + e()−2νπ1π2()ln + e ()2νπ1νπ32ν2ν2() + e ()2νπ1() − 1s(A7) For large ν, the function εν (s ) in (A7) can be written as the following series expansion,= ()ενs − + + 124⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟ + + 121π2νπν2 − 1s e ()−2νπ()O ν2e ()−4νπgiving the following asymptotic value for ν >> 1,= ()ενs − + 1()O ν2e ()−2νπ(A8)Remark A3.3. As mentioned in Remark A3.1 above, some freedom is allowed in the choice of the region δ. For sufficiently large N , we can always use this freedom in δ to ensure that the product ν = N δ is large enough for the remainder in (A8) to be negligible.Inserting (A5) and (A6) into (A4) we thus finally get (note that there are no terms of zeroth and first order in 1/N )= ∆⎛⎝⎜⎜⎞⎠⎟⎟I 212πi e ()/12i s π2s πs N s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟ + i () − s 1()ενs N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3For the region around 3π/2 we can similarly calculate the following correction (note again that there are no terms of zeroth and first order in 1/N ),= ∆⎛⎝⎜⎜⎞⎠⎟⎟I 232πi e ()/32i s π2s πs N s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− + i () − s 1()ενs N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3 Adding the two corrections above to the integral I 2 in (A3) over the step function gives= I 2−2s πs N s ()− + e()/32i s πe ()/12i s π⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟+ + 1s () − s 1()ενs N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3sSimplify the sum of exponentials to a sine function, and define the error factor ΕN (s ) as follows= I 22i 2s N s πs e ()i s π⎛⎝⎜⎜⎞⎠⎟⎟sin 12s π()ΕN s s= ()ΕN s + + 1s () − s 1()ενs N 2⎛⎝⎜⎜⎞⎠⎟⎟O 1N 3(A9)where εν (s ) is given in (A7) and (A8) above.Combining the above result for the integral I 2 with the result in Sect A2 for the integral I 1 ,the contour integral I N can thus finally be written as follows [the remainder in I 1 is negligible compared to the remainder in I 2 from ΕN (s )],= I N − i 2() + s 1N s πs e ()i s π⎛⎝⎜⎜⎞⎠⎟⎟sin 12s π()ΕN s 2i e ()i s π()sin s π() − 12() − 1s ()Γs ()ζs (A10) (A10), (A9), and (A8) derived above thus prove Theorem 4.1 in Sect 4.A PPENDIXB . Calculation of ζN - ζ in closed form(13) and (14) should hold (with other error factors) also for N −> N + 1, i e= ()ζ + N 1s 'πs ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟− + 2() − s 1() + N 1s ()Ε + N 1s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1 + N 1() − 2n 1() − s 1s ⎛⎝⎜⎜⎞⎠⎟⎟cos 2s π()− + 12() − 1s ()Γ + s 1 = ()ζ + N 1s ''− + ()+ N 1() − 1s ()Ε + N 1 − 1s 2s ⎛⎝⎜⎜⎜⎞⎠⎟⎟⎟∑ = n 1 + N 1() − 2n 1()−s () − 1s ()− + 12s ()− 1s where the notation ζN+1(s ) denotes that these expressions for the Riemann zeta-function correspond to truncation at order N + 1 in the above series .Calculate the difference between (13) and (14), respectively, and the above relationships, =− ()ζN s '()ζ + N 1s 'πs ()− + − 2() − s 1N s ()ΕN s 2() − s 1() + N 1s ()Ε + N 1s s () + 12N () − s 1⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 12() − 1s ()Γ + s 1 = − ()ζN s ''()ζ + N 1s ''− + − N ()− 1s ()ΕN − 1s () + N 1() − 1s ()Ε + N 1 − 1s 2s () − 1s () + 12N ()−s () − 1s ()− + 12s Below we now insert the expressions for ΕN and ΕN+1 from (12a) and (12b), and use the value of εν (s ) in (A8). As will be further discussed later, we also temporarily omit theremainders O(1/N 3 ) and O(1/(N +1) 3 ) from ΕN and ΕN+1 in the expressions below Remark B.1. It should be remembered that the error εν (s ) was calculated in Appendix A at(A7) and (A8), and implicitly involved in (7) through (11). This error εν (s ) was thengeneralized to allow arbitrary finite values at (13) and (14) onwards, via (15) and (16), and through Appendix B up to the present point. We thus now here again specialize εν (s ) to be given as in (A8), i e to be equal to –1/24 (cf Remark A3.3).Remark B.2. For sufficiently large N , i e for N >> Im (s ), the differences ζN (s )´- ζN+1(s )´and ζN (s )´´- ζN+1(s )´´ are both smooth, slowly varying functions of N . Their resulting,respective truncation errors will then be one power of 1/N smaller than the differences themselves, as will be shown in the series expansions later. It should be remarked that the individual remainders O(1/N 3 ) and O(1/(N +1) 3 ) in ΕN and ΕN+1, which are omitted in the expressions below, could each easily be several orders of magnitude larger than the small resulting differences ζN (s ) - ζN+1(s ) calculated below. Crucially, however - as will also be shown below - the resulting remainders [corresponding to the actual combination of the remainders O(1/N 3 ) and O(1/(N +1) 3 )] will still be of lower order than these resulting differences ζN (s ) - ζN+1(s ).= − ()ζN s '()ζ + N 1s '= πs ⎛⎝⎜⎜⎞⎠⎟⎟− + − 2() − s 1N s ⎛⎝⎜⎜⎞⎠⎟⎟ − 1124s () − s 1N 22() − s 1() + N 1s ⎛⎝⎜⎜⎞⎠⎟⎟ − 1124s () − s 1() + N 12s () + 12N () − s 1⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 12() − 1s ()Γ + s 1 = − ()ζN s ''()ζ + N 1s ''= − + − N() − 1s ⎛⎝⎜⎜⎞⎠⎟⎟ − 1124s () − s 1N 2() + N 1() − 1s ⎛⎝⎜⎜⎞⎠⎟⎟ − 1124s () − s 1() + N 122s () − 1s () + 12N ()−s () − 1s ()− + 12s In order to study the above two relationships, we make Taylor expansions in 1/N of the factors (N +1) and (1+2N ) with their different exponents. By their very nature, the two differences above are very small. As a consequence, the handling of the terms and theresulting truncation errors in the above two relationships require series expansions of high order to get non-vanishing results. [For the same reason, numerical calculations of the right-hand sides above have to be made using high accuracy (30 digits or more) in order to give correct results]. The leading terms in the series expansions of the two relationships above are= − ()ζN s '()ζ + N 1s ' + 711520πs 4s N ()− s 5⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 22s ()Γ − s 4()O N () − σ6 = − ()ζN s ''()ζ + N 1s ''− + 7() + s 3() + s 2() + s 1N ()− − s 4s − + 12s ()O N ()− − σ5 We now wish to replace ζN +1(s ) in these expressions by the zeta-function itself [this is also how we will be able to convert the truncation errors in the above expressions into remainders relative to the exact zeta-function, and thus reinstate correct resulting remainders in place of those pairs of individual remainders O(1/N 3 ) and O(1/(N +1) 3 ) that we omitted above]. We begin by considering first-order Taylor expansions in 1/N as follows,= − N() − s 4() + N 1() − s 4− + N () − s 5() − s 4()O N () − σ6 = − N ()− − 3s () + N 1()− − 3s − + N ()− − s 4()− − 3s ()O N ()− − σ5and divide the left-hand sides of the two previous results by the left-hand sides of the respective Taylor expansion, and similarly for the right-hand sides, = − ()ζN s '()ζ + N 1s '− N () − s 4() + N 1() − s 4− + 7πs 4s ⎛⎝⎜⎜⎞⎠⎟⎟cos s π()− + 22s ()Γ − s 3⎛⎝⎜⎜⎞⎠⎟⎟O 1 = − ()ζN s ''()ζ + N 1s '' − N ()− − 3s () + N 1()− − 3s − + 7() + s 2() + s 1s − + 12s ⎛⎝⎜⎜⎞⎠⎟⎟O 1 For use in Sect 6, we also equate the quotient of the left-hand sides above to the quotient of the right-hand sides, − ()ζN s '()ζ + N 1s ' − N () − s 4()+ N 1() − s 4 711520πs 4s ⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()− + 22s ()Γ − s 3 _______________________________ = _________________________________________________ + O(1 / N ) − ()ζN s ''()ζ + N 1s '' − N()− − 3s () + N 1()− − 3s 75760() + s 2() + s 1s − + 12s The right-hand side above becomes = RHS + 12πs ()− + 4s 8s ⎛⎝⎜⎜⎞⎠⎟⎟cos 12s π()Γ − s 3() + s 2() + s 1s ()− + 22s ⎛⎝⎜⎜⎞⎠⎟⎟O 1N。

Accurate long-range coefficients for two excited like isotope He atoms He($2 ^1P$)--He($2 ^

a r X i v :0705.1604v 1 [p h y s i c s .a t o m -p h ] 11 M a y 2007Accurate long-range coeﬃcients for two excited like isotope He atoms:He(21P )–He(21P ),He(21P )–He(23P ),and He(23P )–He(23P )J.-Y.Zhang,∗1Z.-C.Yan,1,2D.Vrinceanu,3J.F.Babb,4and H.R.Sadeghpour 41Department of Physics,University of New Brunswick,Fredericton,New Brunswick,Canada E3B 5A32Shanghai United Center for Astrophysics,Shanghai Normal University,100Guilin Road,Shanghai,People’s Republic of China 2002343Theoretical Division,Los Alamos National Laboratory,Los Alamos,New Mexico 87545,USA and4ITAMP,Harvard-Smithsonian Center for Astrophysics,Cambridge,Massachusetts 02138,USA(Dated:February 5,2008)AbstractA general formalism is used to express the long-range potential energies in inverse powers of the separation distance between two like atomic or molecular systems with P symmetries.The long-range molecular interaction coeﬃcients are calculated for the molecular symmetries ∆,Π,and Σ,arising from the following interactions:He(21P )–He(21P ),He(21P )–He(23P ),and He(23P )–He(23P ).The electric quadrupole-quadrupole term,C 5,the van der Waals (dispersion)term C 6,and higher-order terms,C 8,and C 10,are calculated ab initio using accurate variational wave functions in Hylleraas coordinates with ﬁnite nuclear mass eﬀects.A comparison is made with previously published results where available.PACS numbers:32.10.Dk,34.20.CfI.INTRODUCTIONAccurate description of the interactions between two excited atoms(or molecules)at long-range is fundamentally important for studies of molecular excited state spectroscopy[1, 2],associative ionization[3,4],and other collisional processes[5],and is at the heart of several schemes for quantum computation[6,7].At suﬃciently large separations,the mutual electrostatic interaction energy between the two excited atoms can be accurately described using an expansion of the potential energy in inverse powers of the separation distance R. The terms describe the electric quadrupole-quadrupole interaction at order R−5and the instantaneous dipole-dipole(e.g.dispersion)interaction at order R−6[8]and higher order instantaneous multipole-multipole interactions at orders R−8and R−10.Long-range interactions involving few-electron atoms are the only interactions that presently can be rigorously calculated with high accuracy.Diﬀerent levels of approxima-tion are needed for the calculations of long-range forces for alkali-metal and alkaline-earth atoms.[5,9,10].Sizeable discrepancies between various calculations in the literature can occur,as illustrated in the comparisons of C6coeﬃcients,for example,given by Zhang et al.[12]for Li(2p)–Li(2p)and given by Yurova[13]for Na(3p)–Na(3p).For helium,it is possible to perform a highly-accurate ab initio calculation of atomic properties and long-range interaction coeﬃcients.Such results could become benchmarks for eventual ab initio calculations of alkaline-earth atomic interactions.Alkaline-earth and other two-electron ex-cited P atoms are currently being studied as the optimal candidates for frequency-based standards and optical clock experiments[14].We had previously studied the long-range interaction coeﬃcients C n(with n≤10)for all He(nλS)–He(n′λ′S)and He(nλS)–He(n′λ′P)systems of the energetically lowestﬁve states: He(11S),He(23S),He(21S),He(23P)and He(21P)and theﬁnite nuclear mass eﬀects for like isotopes[15,16,17].In this work,we present results for more complicated set of interactions between two like isotope helium atoms with P symmetries.Degenerate perturbation theory is needed to derive the interaction terms for some of the terms.Section II introduces a general formalism for calculating dispersion coeﬃcients between two like atomic or molecular systems of P symmetry.Section III presents numerical results of dispersion coeﬃcients C5, C6,C8,and C10for the following three systems He(21P)–He(21P),He(21P)–He(23P),and He(23P)–He(23P).II.FORMULATIONIn this work,atomic units are used throughout.At large distances R between two atoms a and b,the Coulomb interaction[18],treated as a perturbation to the two isolated atoms, is∞ ℓ=0∞ L=0VℓLV=√where M a =M b =1are the magnetic quantum numbers,αis the normalization factor,and βdescribes the symmetry due to the exchange of two initial states Ψn a and Ψn b .If twoatoms are both in the same P state,thenα=√R 5,(7)where,after some angular momentum algebra,one gets (see also Ref.[11]),C 5(∆,β)=−A 1+βA 2,(8)A 1=4π25| Ψn a (σ)||iQ i σ2i Y 2(ˆσi )||Ψn b (σ) |2.(10)The second-order energy isV(2)=−n s n t ′ L s M sL t M t| Ψ(0)(∆)|V |χn s (L s M s ;σ)ωn t (L t M t ;ρ) |2E n s n t −E (0)n a n b,(12)withB 1=| Ψn a (M a ;σ)Ψn b (M b ;ρ)|V |χn s (L s M s ;σ)ωn t (L t M t ;ρ) |2,(13)B 2= Ψn a (M a ;σ)Ψn b (M b ;ρ)|V |χn s (L s M s ;σ)ωn t (L t M t ;ρ)× Ψn a (M a ;ρ)Ψn b (M b ;σ)|V |χn s (L s M s ;σ)ωn t (L t M t ;ρ) .(14)After applying the Wigner-Eckart theorem,we haven s n t ′ L s M sL t M tB 1R ℓ+L +ℓ′+L ′+2,(15)withD1(ℓ,L,ℓ′,L′)=12× n s n t¯g n s;n a n a(L s,1,1,ℓ,ℓ′)¯g n t;n b n b(L t,1,1,L,L′)(ℓ,ℓ′)(2ℓ+1)2(2L1+1)| Ψn a(L1;σ)||i Q iσℓi Yℓ(ˆσi)||χn s(L s;σ) |2.(20) Similarly,we haven s n t′ L s M s L t M t B2Rℓ+L+ℓ′+L′+2,(21)D2(ℓ,L,ℓ′,L′)=1×1ℓL s−M a µM a −µ1L ′L t−M a M a −M b −µM b +µ×1L L t−M b−µM b +µ1ℓ′L s −M bM b −M a +µM a −µ,(23)F 2(ℓ,L,ℓ′,L ′,L a ,L b ,L s ,L t )=9π(∆E n s ,n a +∆E n t ,n b )R 2n,(25)where the dispersion coeﬃcients C 2n (∆,β)are deﬁned byC 2n (∆,β)=ℓ,L,ℓ′,L ′≥1ℓ+L +ℓ′+L ′+2=2n[D 1(ℓ,L,ℓ′,L ′)+βD 2(ℓ,L,ℓ′,L ′)].(26)B.ΠstateFor the Πstate,the zeroth-order wave function is in the following formΨ(0)(Π,β,γ)=α2[Ψn a (M a ;ρ)Ψn b (M b ;σ)+γΨn a (M b ;ρ)Ψn b (M a ;σ)],(27)where β=±1,M a =0,and M b =1.If n a =n b ,α=√E n s n t −E (0)n a n b.(29)Introducing a function g o deﬁned byg o (M a ,M b ,M c ,M d ,ℓ,L,L s ,L t ,ℓ′,L ′)=(−1)L +L ′(ℓ,L,ℓ′,L ′)1/2µµ′M s M tK µℓL K µ′ℓ′L ′×1ℓL s−M aµM s1L L t−M b−µM t1ℓ′L s −M cµ′M s1L ′L t −M d −µ′M t,(30)one can write V (2)(Π,β,γ)asV(2)(Π,β,γ)=−n ≥3C 2n (Π,β,γ)16L s L tf 1γ(ℓ,L,L s ,L t ,ℓ′,L ′)G 1+f 2γ(ℓ,L,L s ,L t ,ℓ′,L ′)G 2,(33)f 1γ(ℓ,L,L s ,L t ,ℓ′,L ′)=12(1+γ2)[g o (0,1,1,0,ℓ,L,L s ,L t ,ℓ′,L ′)+g o (1,0,0,1,ℓ,L,L s ,L t ,ℓ′,L ′)]+γg o (0,1,0,1,ℓ,L,L s ,L t ,ℓ′,L ′)+γg o (1,0,1,0,ℓ,L,L s ,L t ,ℓ′,L ′),(35)G 1=n s n t′ ¯g n s ;n a n a (L s ,1,1,ℓ,ℓ′)¯g n t ;n b n b(L t ,1,1,L,L ′)(∆E n s ,n a +∆E n t ,n b )|∆E n s ,n a ∆E n t ,n b |,(36)andG 2=βn s n t′¯g n s ;n a n b (L s ,1,1,ℓ,ℓ′)¯g n t ;n b n a (L t ,1,1,L,L ′)|∆E n s ,n a ∆E n s ,n b ∆E n t ,n a ∆E n t ,n b |+¯g n s ;n b n a (L s ,1,1,ℓ,ℓ′)¯g n t ;n a n b (L t ,1,1,L,L ′)|∆E n s ,n a ∆E n s ,n b ∆E n t ,n a ∆E n t ,n b |.(37)From the above expressions,it is clear that f 1γ=γf 2γwhen γ=±1and G 2=βG 1when γ=0.C.ΣstateFor theΣstate,the possible zeroth-order wave functions for the combined system a-b areΨ(0)(Σ,β)=α2[Ψn a(M a;σ)Ψnb(M b;ρ)+βΨn a(M a;ρ)Ψnb(M b;σ)],(38)andΨ(0)(Σ,β,γ)=α2[Ψn a(M a;ρ)Ψnb(M b;σ)+γΨn a(M b;ρ)Ψnb(M a;σ)].(39)In theΨ(0)(Σ,β)state we have M a=M b=0,while in theΨ(0)(Σ,β,γ)state,M a=−M b= 1,andγ=±1.When n a=n b,β=0andα=√ForΨ(0)(Σ,β)andΨ(0)(Σ,β,1),the matrix elements of the Coulomb interaction V areΨ(0)(Σ,β)|V|Ψ(0)(Σ,β) =−4C5(∆,β)2C5(∆,β).(46)R5Theﬁrst-order energies are obtained by the diagonalization of the following matrix:.(47)−4C5(∆,β)√√2They areλ1=0,(48)6C5(∆,β)λ2=−√221,(53)R2nwhereC2n(Σ,β;λi)= ℓ,L,ℓ′,L′≥1ℓ+L+ℓ′+L′+2=2n Dλi(ℓ,L,ℓ′,L′),(54)Dλi (ℓ,L,ℓ′,L′)=3α22g o(−1,1,−1,1,ℓ,L,L s,L t,ℓ′,L′)+ 12g o(1,−1,−1,1,ℓ,L,L s,L t,ℓ′,L′)+12[g o(−1,1,−1,1,ℓ,L,L s,L t,ℓ′,L′)−g o(−1,1,1,−1,ℓ,L,L s,L t,ℓ′,L′)−g o(1,−1,−1,1,ℓ,L,L s,L t,ℓ′,L′)+g o(1,−1,1,−1,ℓ,L,L s,L t,ℓ′,L′)].III.CALCULATIONS AND RESULTSIn the center-of-mass frame,the Hamiltonian of a helium atom can be written in the formH=−12µe∇2r2−1r1−2r12,(59)where m n is the nucleus mass,µe is the reduced mass between the electron and the nucleus, r1and r2are the position vectors of the two electrons relative to the nucleus,and r12isthe distance between them.To calculate dispersion coeﬃcients C n,we variationally evalu-ate energy eigenvalues and corresponding eigenfunctions of the initial states He(21P)and He(23P)with the correlated Hylleraas basis set{r i1r j2r k12e−αr1−βr2Y LMℓ1ℓ2(ˆr1,ˆr2)},(60) where Y LM(ˆr1,ˆr2)is the coupled spherical harmonics for the two electrons forming a common ℓ1ℓ2eigenstate of L2and L z,andαandβare two nonlinear parameters,which are optimized by ﬁrst calculating analytically theﬁrst-order derivatives of the variation energy with respect to these parameters and then using Newton’s method toﬁnd their roots.The basis set includes all combinations of i≥ℓ1,j≥ℓ2,and k≥0with i+j+k≤Ω,whereΩis an integer controlling the size of the basis set.Then we generate the spectra of the intermediate states for the S,P,D,F,and G symmetries by diagonalizing directly the Hamiltonian of the helium atom in chosen basis sets.In addition,we need to transform the2ℓ-pole transition operator Tℓµ(σ),deﬁned in Eq.(3),into the center-of-mass coordinates and calculate the reduced matrix elements of dipole,quadrupole,octupole,and hexapole transition operators (for details,refer to Ref.[19]).Table I shows the convergence study of the nonrelativistic energy of the∞He(23P)state with the increase of the size of basis pared to the value of Drake et al.[20],our result is accurate to about15digits.Table II gives the convergence pattern of C6(∆,0), C6(Π,+,0),and C6(Σ,0;λ1)for∞He(23P)–∞He(23P)as the sizes of basis sets,including the initial state and the three intermediate states,increase progressively.Table III presents the long-range interaction coeﬃcients C5,C6,C8,and C10for the He(21P)-He(21P)system.Table IV shows C5,C6,C8,and C10for the He(21P)-He(23P) system.Table V lists C5,C6,C8,and C10for the He(23P)-He(23P)system.We note that, for the three He(n P)–He(n′P)systems,C5(Π,−,β),C5(Σ,β;λ1),and C5(Σ,β;λ3)are zero, C5(Π,+,β)and C6are positive,and both C5(∆,β)and C5(Σ,β;λ2)are negative.For the Ψ(0)(Σ,β;λ3)states,C8and C10are negative in the three tables.Ovsyannikov obtained expressions for the C6(dispersion)coeﬃcients between two ex-cited atoms[9]and evaluated the He(23P2)–He(23P2)coeﬃcients using the atomic dynamic polarizability obtained with a model potential for the He(23P)atom.The diagonal elements of the long-range interaction were given in the jj representation;after transformation to the LS representation[13]the results of Ref.[9]can be compared with ours.The one signiﬁ-cant discrepancy is between our results for the summed C6(Σ,0;λ)and that of Ref.[9].In table VI we compare the transformed coeﬃcients of Ref.[9]with the present calculations.To the best of our knowledge,there are no other published results for the dispersion coeﬃcients for interaction between He(21P)-He(21P)and He(21P)-He(23P).AcknowledgmentsThis work is supported by the Natural Sciences and Engineering Research Council of Canada,by the Canadian computing facilities of ACEnet,SHARCnet,and WestGrid,by DOE and by NSF through a grant for the Institute of Theoretical Atomic,Molecular and Optical Physics(ITAMP)at Harvard University and Smithsonian Astrophysical Observa-tory.JYZ and ZCY would like to thank ITAMP for its hospitality during their visits.ZCY would also like to acknowledge the support by NSC of ROC during his visit at the Institute of Atomic and Molecular Sciences,Academia Sinica.[1]O.Dulieu,B.L´e vy,S.Magnier,F.Masnou-Seeuws,and li´e,Phys.Rev.Lett.76,2858(1996).[2] C.Boisseau,I.Simbotin,and R.Cˆo t´e,Phys.Rev.Lett.88,133004(2002).[3]R.W.Heather,and P.S.Julienne,Phys.Rev.A47,1887(1993).[4] A.Amelink,K.M.Jones,P.D.Lett,P.van der Straten,and H.G.M.Heideman,Phys.Rev.A61,042707(2000).[5] A.Derevianko,S.G.Porsev,S.Kotochigova,E.Tiesinga,P.S.Julienne,Phys.Rev.Lett.90,063002(2003).[6]K.Singer,J.Stanojevic,M.Weidem¨u ller,and R.Cˆo t´e,J.Phys.B38,S295(2005).[7]T.G.Walker and M.Saﬀman,J.Phys.B38,S309(2005).[8]T.Y.Chang,Rev.Mod.Phys.39,911(1967).[9]V.D.Ovsyannikov,Opt.Spektrosk.53,600(1982)[Opt.Spectrosc.(USSR)53,357(1982).[10]M.Marinescu Phys.Rev.A56,4764(1997).[11] B.Zygelman,A.Dalgarno,and R.D.Sharma49,2587(1994).[12]J.-Y.Zhang,J.Mitroy,and M.W.J.Bromley,Phys.Rev.A75,042509(2007).[13]I.Yu.Yurova,Phys.Rev.A65,032726(2002).[14]/itamp/UltraColdII/UltraColdII.html.[15]J.-Y.Zhang,Z.-C.Yan,D.Vrinceanu,and H.R.Sadeghpour,Phys.Rev.A71,032712(2005).[16]J.-Y.Zhang,Z.-C.Yan,D.Vrinceanu,J.F.Babb,and H.R.Sadeghpour,Phys.Rev.A73,022710(2006).[17]J.-Y.Zhang,Z.-C.Yan,D.Vrinceanu,J.F.Babb,and H.R.Sadeghpour,Phys.Rev.A,74,014704(2006).[18]Z.-C.Yan,J.F.Babb,A.Dalgarno,and G.W.F.Drake,Phys.Rev.A54,2824(1996).[19]J.-Y.Zhang and Z.-C.Yan,J.Phys.B37,723(2004).[20]G.W.F.Drake,Can.J.Phys A80,1195(2002).TABLE I:Convergence study for the nonrelativistic energyof He(23P)with the inﬁnite nuclear mass.N denotes thenumber of terms in the basis set.Units are atomic units.ΩN E(Ω)12910–2.133164190779194131120–2.133164190779246141360–2.133164190779279151632–2.1331641907792812161892–2.1331641907792826Drake[20]–2.133164190779283202(5)TABLE II:Convergence study of C6(∆,0),C6(Π,+,0),and C6(Σ,0;λ1),in atomic units,for∞He(23P)–∞He(23P).N23P,N3S,N(pp)3P,and N3D denote respectively the sizes ofbases for the initial state and the three intermediate statesof symmetries3S,(pp)3P,and3D.N23P N3S N(pp)3P N3D C6(∆,0)C6(Π,+,0)C6(Σ,0;λ1) 136056012308534083.026993802324.937567247296.82132476 1632680143010714083.026997992324.937572247296.82133048 1938816165013234083.026998662324.937572917296.82133149units,for the He(21P)-He(21P)system.C n∞He(21P)–∞He(21P)4He(21P)–4He(21P)3He(21P)–3He(21P) C5(∆,0)−227.26160604(2)−227.31888382(1)−227.33762037(2) C5(Π,+,0)909.04642409(1)909.27553527(2)909.35048146(3) C5(Π,−,0)000C5(Σ,0;λ1)000C5(Σ,0;λ2)−1363.56963613(1)−1363.91330289(1)−1364.02572224(5) C5(Σ,0;λ3)000C6(∆,0)5578.63(2)5584.99(1)5587.07(1)C6(Π,+,0)3606.63(2)3610.56(2)3611.84(1)C6(Π,−,0)10831.74(2)10844.25(2)10848.35(2)C6(Σ,0;λ1)9682.78(1)9693.98(2)9697.63(2)C6(Σ,0;λ2)1025.14(2)1032.23(2)1034.58(3)C6(Σ,0;λ3)1966.062(1)1968.141(1)1968.821(1)C8(∆,0)1556.9(6)1458.9(7)1426.6(6)C8(Π,+,0)−170489.4(5)−170548.7(5)−170567.9(3)C8(Π,−,0)1315208(3)1316393(2)1316781(2)C8(Σ,0;λ1)1106570(2)1107553(2)1107874(2)C8(Σ,0;λ2)1788288(2)1790861(2)1791704(2)C8(Σ,0;λ3)−16971.98(4)−17030.68(3)−17049.91(3)C10(∆,0)3.61343(3)×1063.61541(2)×1063.61607(3)×106 C10(Π,+,0)5.163864(4)×1075.168052(3)×1075.169423(3)×107 C10(Π,−,0)1.7334869(4)×1081.7349273(2)×1081.7353990(4)×108 C10(Σ,0;λ1)2.0453997(5)×1082.0471586(3)×1082.0477345(5)×108 C10(Σ,0;λ2)7.6324701(1)×1087.6394035(3)×1087.6416728(2)×108 C10(Σ,0;λ3)−2.992224(2)×106−2.996113(3)×106−2.997383(2)×106units,for the He(21P)-He(23P)system.Mass∞He(21P)–∞He(23P)4He(21P)–4He(23P)3He(21P)–3He(23P) C5(∆,±)−189.519232605(3)−189.513470009(3)−189.511579458(2) C5(Π,+,±)758.07693042(3)758.05388004(2)758.04631785(3) C5(Π,−,±)000C5(Σ,±;λ1)000C5(Σ,±;λ2)−1137.11539564(3)−1137.08082006(2)−1137.06947676(2) C5(Σ,±;λ3)000C6(∆,±)5186.93(2)5190.06(3)5191.91(2)C6(Π,+,±)2990.19(1)2992.03(2)2992.640(2)C6(Π,−,±)10353.76(2)10359.97(5)10362.05(2)C6(Σ,±;λ1)9245.11(2)9250.65(3)9252.52(3)C6(Σ,±;λ2)6162.84(4)6165.27(3)6166.13(4)C6(Σ,±;λ3)1505.135(1)1506.081(1)1506.390(1)C8(∆,±)−41757.6(6)−41832.7(4)−41856.3(4)C8(Π,+,±)−88728.8(2)−88801.9(1)−88825.1(2)C8(Π,−,±)993884(3)994253(2)994375(2)C8(Σ,±;λ1)843530(3)843832(2)843931(2)C8(Σ,±;λ2)2139396(4)2140392(3)2140712(2)C8(Σ,±;λ3)−11571.12(3)−11605.17(2)−11616.32(3)C10(∆,±)2.32333(4)×1062.32313(3)×1062.32312(1)×106 C10(Π,+,±)5.236817(3)×1075.238065(4)×1075.238463(1)×107 C10(Π,−,±)1.224688(2)×1081.224994(2)×1081.225094(1)×108 C10(Σ,±;λ1)1.435423(2)×1081.435806(2)×1081.435933(1)×108 C10(Σ,±;λ2)5.737754(2)×1085.739452(2)×1085.740007(2)×108 C10(Σ,±;λ3)−2.018823(3)×106−2.020165(2)×106−2.020604(2)×106units,for the He(23P)-He(23P)system.Mass∞He(23P)–∞He(23P)4He(23P)–4He(23P)3He(23P)–3He(23P) C5(∆,0)−158.0449076079(7)−157.9954762726(5)−157.9793027289(2) C5(Π,+,0)632.179630432(3)631.981905089(1)631.917210917(2) C5(Π,−,0)000C5(Σ,0;λ1)000C5(Σ,0;λ2)−948.269445647(4)−947.972857636(3)−947.875816375(3) C5(Σ,0;λ3)000C6(∆,0)4083.0269989(3)4082.9351409(1)4082.9049629(7) C6(Π,+,0)2324.9375732(4)2325.2001488(4)2325.2859848(3) C6(Π,−,0)8172.742842(3)8172.314300(1)8172.173845(3)C6(Σ,0;λ1)7296.821336(5)7296.447426(2)7296.324875(5)C6(Σ,0;λ2)8367.5905(5)8361.2048(2)8359.1157(4)C6(Σ,0;λ3)1159.1243658(5)1159.3780657(5)1159.4610336(4) C8(∆,0)−30170.71(6)−30168.72(8)−30168.10(6)C8(Π,+,0)17083.8(2)17044.4(2)17031.5(3)C8(Π,−,0)726869.3(2)726728.9(2)726682.8(1)C8(Σ,0;λ1)618334.9(1)618201.6(2)618157.8(1)C8(Σ,0;λ2)1835912.3(2)1835108.6(4)1834846(1)C8(Σ,0;λ3)−8209.2(2)−8229.3(3)−8236.16(8)C10(∆,0)1537493(3)1536725(3)1536474(3)C10(Π,+,0)3.656172(2)×1073.653559(2)×1073.652704(2)×107 C10(Π,−,0)8.4139100(8)×1078.410605(1)×1078.409524(2)×107 C10(Σ,0;λ1)9.846488(2)×1079.842827(2)×1079.841629(2)×107 C10(Σ,0;λ2)3.9464965(4)×1083.94483100(3)×1083.9442865(4)×108 C10(Σ,0;λ3)−1.378107(3)×106−1.378199(2)×106−1.378225(5)×106TABLE VI:Comparison of the present results with the avail-able results of Ref.[9]for the C6coeﬃcients of He(23P2)–He(23P2)with inﬁnite nuclear mass.The relation betweenthe present results(LHS)and the results from Ref.[9](RHS)are given in theﬁrst column.The symbols for the dispersioncoeﬃcients on the RHS represent values C6(M A,M B)for twoatoms in the total angular momentum states J A=J B=2and quantization axis along the inter-nuclear axis.Terms Present Ref.[9] C6(∆,0)=C6(2,2)40834056 13 3i=1C6(Σ,0;λi)=4C6(0,2)+43C6(1,2)+7。

13 Nov 2012 Equipment Log

Equipment Log
Equipment Log Scion ID# YYXXX 12001 12 12008 12009 12010 12011 12012 12013 12014 13001 13002 13003 13004 13005 13006 13007 13008 13009 13010 13011 13012 13013 13014 13015 13016 13017 13018 13019 13020 13021 13022 13023 13024 13025 13026 13027 13028 13029 13030 13031 13032 13033 13034 13035 13036 13037 14001 14002 14003 14004 14005 14006 14007 14008 14009 14010 14011 14012 Description Model # Serial # 122287762 284907 HR11122057 59408 N/A N/A N/A N/A N/A N/A C010096 W5022S1130273 4ZW1 671680 C47118 122644038 0012-0140 332 0601 0488 332 0601 0583 332 0902 0052 000181 02251023 8746013 B013179 C010074 C010106 C010112 E00084308 N/A N105855ZBV N145868YKV N96136583 ACS-AM503-PS 0001188 027811 031937 224242 260510 3257810 3257823 3797281 77140785 84380233 85070010 LM4B RLC07124005E 895A0C400178/181 895A0C400178/181/50LB0020 85300276 020101691 118F13125 NA NA NA NA NA NA NA NA NA NA NA 4798 Manufacturer Control Company Omegadyne Tree Mitutoyo Neiko C3 Fluke Fluke Fluek N/A Tektronix OWON N/A Omagadyne GEI Instrnational Inc Traceable BK Precision BK Precision BK Precision BK Precision Schaffner Instrument Global Specialties Fluke Tektronix Tektronix Tektronix Tektronix Xantrex Tektronix Fluke Fluke BK Precision Tektronix Leader TPI TPI Global Specialties Fluke Leader Leader Leader Fluke Fluke Fluke Meyer Branson CHATILLON CHATILLON Fluke Wavetek BK Precision Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Scion Engineering Troemner Location Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Biohazard Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Engineering Lab Calibration Customer Owned? Frequency No 2 Years No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No N/A No 2 Years No N/A No N/A No N/A No N/A No 1 Year No N/A No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No N/A No 1 Year No 1 Year No 1 Year No N/A No 1 Year No N/A No 1 Year No 1 Year No N/A No 1 Year No N/A No N/A No N/A No 1 Year No 1 Year No 1 Year No N/A No N/A No 1 Year No 1 Year No 1 Year No 1 Year No 1 Year No N/A No N/A No N/A No N/A No N/A No N/A No N/A No N/A No N/A No N/A No N/A No 1 Year Maint Frequency 2 Years N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A 2 Years N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A N/A

2013国标清单

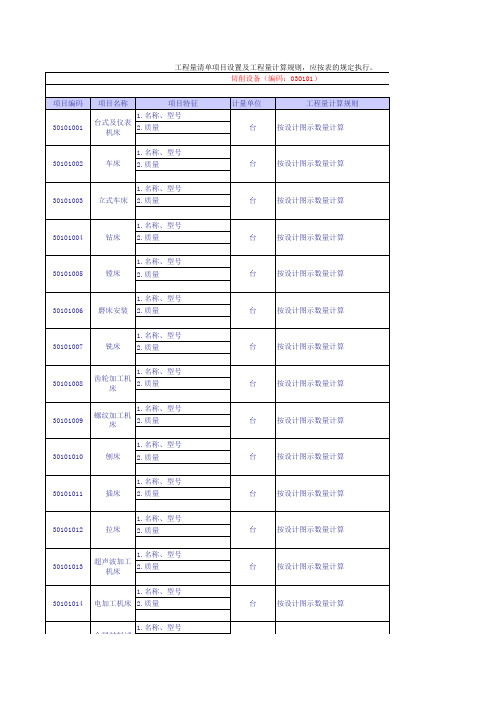
验机械工程量清单项目设置及工程量计算规则，应按表的规定执行。

工程量清单项目设置及工程量计算规则，应按表的规定执行。

塔器（柜）验工程量清单项目设置及工程量计算规则，应按表的规定执行。

统校正处理设备机安装炉制作安装罐制作安装件，以几何尺寸展开面积计算，不扣孔洞所占面积，并增加各部位搭接和对接垫板的金属质量。

@@2.不同的板幅应按规定调整其金属质量。

@@3.油罐附件以不同的种类和规格分别计算质量按设计图纸的质量计算，包括内件及附件的质量长米计算，不扣除阀门、管件所占长度，遇弯头时，按两管交叉的中心线交点计算。

方形补偿器以其所占长度按管道安装工程量计算制安装长米计算，不扣除阀门、管件所占长度，遇弯头时，按两管交叉的中心线交点计算。

方形补偿器以其所占长度按管道安装工程量计算管的中心线交点计算。

方形补偿器以其所占长度按管道安装工程量计算帽、方形补偿器弯头、管道上仪表一次部件、仪表温度计扩大管制作安装等 @@注2：管件压力试包括弯头、三通、四通、异径管管件帽、方形补偿器弯头、管道上仪表一次部件、仪表温度计扩大管制作安装等 @@注2：管件压力试验、吹扫、清洗、脱脂、除锈、包括弯头、三通、四通、异径管门、仪表流量计均按阀门安装@@注2：减压阀直径按高压侧计算@@注3：电动阀门包括电动机安装按设计图示数量计算@@注1：各种门形式补偿器（除方形补偿器外）、仪表流量计均按阀门安装@@注2：减压阀直径按高压侧计算对焊法兰法兰、焊接盲板和封头按法兰安装计算，但法兰盲板不计安装工程量 @@注2：不锈钢、有色金属材质的焊环活动法兰按翻边活动法兰安装计算。

安全知识考查

C、路面附着力增大D、制动性能没有变化9泥泞道路对安全行车的主要影响是什么？A、行驶阻力变小B讨论B、车轮极易滑转和侧滑C、能见度低，视野模糊D、路面附着力增大10水淹路面影响行车安全，不易通行的原因是什么？A、无法观察到暗坑和凸起的路面A讨论B、路面附着力增大C、能见度低，视野模糊D、日光反射阻挡视线11山区道路对安全行车的主要影响是什么？A、道路标志少C讨论B、交通情况单一C、坡长弯急，视距不足D、车流密度大12行车中突遇对方车辆强行超车，占据自己车道，正确的做法是什么？A、加速行驶B讨论B、尽可能减速避让、直至停车C、保持原车速行驶D、挡住其去路对讨论13夜间行车，驾驶人视距变短，影响观察，同时注意力高度集中，易产生疲劳。

对讨论14冰雪道路行车，由于积雪对光线的反射，极易造成驾驶人目眩而产生错觉。

对讨论15在冰雪道路上行车时，车辆的稳定性降低，加速过急时车轮极易空转或溜滑。

16在泥泞路上制动时，车轮易发生侧滑或甩尾，导致交通事故。

对讨论17漫水道路行车时，应挂高速档，快速通过。

错讨论错讨论18行车中突遇对向车辆强行超车，占据自己车道时，可不予避让，迫使对方让路。

19行车中遇有前方发生交通事故，需要帮助时，应怎样做？C讨论A、尽量绕道躲避B、立即报警，停车观望C、协助保护现场，并立即报警D、加速通过，不予理睬20行车中遇交通事故受伤者需要抢救时，应怎样做？A、及时将伤者送医院抢救或拨打急救电话A讨论B、尽量避开，少惹麻烦C、绕过现场行驶D、借故避开现场21行车中遇到对向来车占道行驶，应怎样做？A、紧靠道路中心行驶B讨论B、主动给对方让行C、用大灯警示对方D、逼对方靠右行驶22行车中发现前方道路拥堵时，应怎样做？A、寻找机会超越前车C讨论B、从车辆空间穿插通过C、减速停车，依次排队等候D、鸣喇叭催促23会车中遇到对方来车行进有困难需借道时，应怎样做？A、不侵占对方道路，正常行驶D讨论B、示意对方停车让行C、靠右侧加速行驶D、尽量礼让对方先行24行车中遇到后方车辆要求超车时，应怎样做？A、及时减速、观察后靠右行驶让行A讨论B、保持原有车速行驶C、靠右侧加速行驶D、不让行25驾驶人在超车时，前方车辆不减速、不让道，应怎样做？A、连续鸣喇叭加速超越C讨论B、加速继续超越C、停止继续超车D、紧跟其后，伺机再超26驾驶人在行车中经过积水路面时，应怎样做？A、特别注意减速慢行A讨论B、迅速加速通过C、保持正常车速通过D、低档加速通过27发现前方道路堵塞，正确的做法是什么？D讨论A、继续穿插绕行B、选择空当逐车超越C、鸣喇叭示意前方车辆快速行驶D、按顺序停车等候28车辆在拥挤路段低速行驶时，遇其他车辆强行插队，应怎样做？A、鸣喇叭警告，不得进入C讨论B、加速行驶，紧跟前车，不让其进入C、主动礼让，确保行车安全D、挤靠“加塞”车辆，逼其离开29当驾驶车辆行经两侧有行人且有积水的路面时，应怎样做？A、加速通过C讨论B、正常行驶C、减速慢行D、连续鸣喇叭30当驾驶车辆行经两侧有非机动车行驶且有积水的路面时，应怎样做？A、减速慢行A讨论B、正常行驶C、加速通过D、连续鸣喇叭对讨论31一个合格的驾驶人，不仅表现在技术的娴熟上，更重要的是应该具有良好的驾驶行为习惯和道德修养。

option hedging for small investors under liquidity costs

Finance Stoch(2010)14:317–341DOI10.1007/s00780-009-0116-xOption hedging for small investors under liquidity costsUmutÇetin·H.Mete Soner·Nizar TouziReceived:8September2006/Accepted:6December2008/Published online:11December2009©Springer-Verlag2009Abstract Following the framework ofÇetin et al.(Finance Stoch.8:311–341,2004), we study the problem of super-replication in the presence of liquidity costs under additional restrictions on the gamma of the hedging strategies in a generalized Black–Scholes economy.Weﬁnd that the minimal super-replication price is different from the one suggested by the Black–Scholes formula and is the unique viscosity solution of the associated dynamic programming equation.This is in contrast with the results ofÇetin et al.(Finance Stoch.8:311–341,2004),whoﬁnd that the arbitrage-free price of a contingent claim coincides with the Black–Scholes price.However,inÇetin et al.(Finance Stoch.8:311–341,2004)a larger class of admissible portfolio processes is used,and the replication is achieved in the L2approximating sense.Keywords Super-replication·Liquidity cost·Gamma process·Parabolic majorant·PDE valuationMathematics Subject Classiﬁcation(2000)91B28·35K55·60H30This research is part of the Chair Financial Risks of the Risk Foundation sponsored by SociétéGénérale,the Chair Derivatives of the Future sponsored by the Fédération Bancaire Française,andthe Chair Finance and Sustainable Development sponsored by EDF and Calyon.Also theﬁrst two authors thank the European Science Foundation for its support through the AMaMeF program.U.Çetin( )London School of Economics,London,UKe-mail:u.cetin@H.M.SonerDepartment of Mathematics,ETH Zurich,Zurich,Switzerlande-mail:mete.soner@math.ethz.chN.TouziCentre de Mathématiques Appliquées,Ecole Polytechnique,Paris,Francee-mail:touzi@cmap.polytechnique.fr318U.Çetin et al. JEL Classiﬁcation C61·G13·D521IntroductionThe Black–Scholes methodology for the pricing and hedging of options requires the market to be frictionless and competitive.In other words,traders can trade any quan-tity of the asset without changing its price,and the trade is subject to no transaction costs and restrictions.There have been numerous works to relax these assumptions as it is now well known that the markets do not operate frictionlessly and are not perfectly competitive(see e.g.[2,3,11–14,17],and[20]).Relaxation of both the frictionless and competitive market hypotheses introduces the notion of liquidity risk.Roughly speaking the liquidity risk is the additional risk due to the timing and size of a trade.Recently,several authors have proposed a num-ber of methods to incorporate the liquidity risk into asset pricing theory(see[1,4–6], and[26]).The common characteristic of all these works is that the liquidity risk ap-pears as some nonlinear transaction cost which appears due to the imbalance between supply and demand in theﬁnancial market,which is relevant if an agent is attempting to trade large volumes in a short time.In the literature dealing with the modeling of“liquidity risk”one can clearly iden-tify two different approaches.In theﬁrst approach,the modeler concentrates on the effects of the large trader’s portfolio on the price of the stock(see,e.g.,[17,18, 25,27,28]).The authors postulate a feedback function that governs the dependence of the equilibrium stock price on the portfolio actions of the large trader.We call this class of models“models of feedback effects”.The second class of models,e.g., [4–6,26],ignores the feedback effects of the trades and concentrates on the equaliza-tion of supply and demand locally in time so that trade volume does not have a lasting impact on the asset price.Consequently the wealth process of a trader in an illiquid market can be decomposed into two components:One comes from the gains/losses due to the changes in the fundamental value of the stock,which does not depend on the history of the trades,and the other is the liquidity cost incurred over time due to the changes in the position.In other words,this class of models studies the behavior of price-taking traders in markets where the change of one’s position has additional liquidity costs.In this work we stay within the framework of the second class of models.In partic-ular,we follow the model suggested byÇetin at al.[4],who introduced the so-called “supply curve”to model the asset price as a function of size and time.Starting with the given supply curve for,say,the stock,the authors show in[4]that the existence of liquidity costs makes trading strategies with inﬁnite quadratic variation infeasible since they incur inﬁnite liquidity costs.One important consequence of their model-ing is that continuous trading strategies ofﬁnite variation incur no liquidity costs; thus,the market is approximately complete(in an L2-sense)if there exists a unique equivalent martingale measure for the“marginal price process”(see[4]for details). In particular,they show that in a Black–Scholes type economy with liquidity costs, the price of an option is given by the standard Black–Scholes formula and the approx-imate hedging strategy can be obtained by some appropriate averaging of the Black–Scholes hedge(see[5]for some further results and numerical and empirical studies).Options with liquidity cost319 On the other hand,the liquidity model of[4]produces a nonzero liquidity pre-mium for options when considered in discrete time(see[6]and results therein).If one looks at the self-ﬁnancing condition in[4]in continuous time,which we recall in Sect.2,one notices that the self-ﬁnancing condition is deﬁned to be the limit of the self-ﬁnancing conditions in discrete time as the time step tends to zero.The discrete-time version of the self-ﬁnancing condition of[4]is very natural since the only assumption on the liquidity cost,other than measurability,is that the bigger the position to liquidate,the larger is the liquidity cost.Therefore,one naturally wonders what happens to the liquidity premium when one passes to the continuous-time limit as it is shown by[4]that the pricing formulas for the contingent claims in their model coincide with those in the frictionless markets.We see this situation as a paradox of the liquidity model of[4]and argue in this paper that the absence of the liquidity premium is linked to the choice of the set of admissible strategies and show that one canﬁnd a nonzero liquidity premium in continuous time for an appropriately deﬁned set of admissible strategies.The correct choice of an admissible set of strategies is crucial even in frictionless markets.Indeed,in models of markets with no friction in discrete time,no condi-tions,other than adaptability,need to be imposed on the trading strategies in order to solve the problems of pricing,hedging,and utility maximization.However,as soon as we consider the continuous-time limit of these models,the price of any contin-gent claim becomes unbounded from below(i.e.,−∞),and the value function of the utility maximization problem will typically be unbounded(i.e.,∞)as one can cre-ate arbitrage opportunities due to the existence of doubling strategies.It is now well known that this paradox can be solved by imposing certain restrictions on the trad-ing strategies,such as certain integrability or lower bound assumptions.The notion of admissibility in frictionless markets is now well understood as an integral part of ﬁnancial modeling.We believe that the apparent paradox in the model of[4]can be solved in a similar way by an appropriate choice of an admissibility condition in illiq-uid markets.The main purpose of this paper is to deﬁne a convenient set of admissible strategies so that the liquidity cost does not vanish in the continuous-time limit.This is achieved by placing constraints on the dynamics of the trading strategies and their corresponding gamma processes as in[7].In a recent paper,Gökay and Soner[19] showed that the continuous-time limit of the corresponding binomial model yields exactly the same pricing equation as in this paper.Since the trading strategies in the binomial model are not restricted,the convergence result of[19]supports our choice of admissible strategies.The restrictions that we place on the trading strategies in this paper can be seen as a relaxation of the restrictions in[21].First of all,we allow a trading strategy to have inﬁnite variation.More precisely,the admissible trading strategies form a larger subset of semimartingales(see(2.2)).As seen,theﬁnite-variation part of a trading strategy consists of a pure-jump component and an absolutely continuous compo-nent.The remaining inﬁnite-variation part is an integral with respect to the marginal price process of the stock,which is a martingale since we work under the unique risk-neutral measure for the marginal price process.The integrand in the absolutely continuous part of the trading strategy can be viewed as the rate of change of the trading strategy with respect to time,while the integrand in the inﬁnite-variation part320U.Çetin et al. can be seen as the rate of change with respect to the changes in the stock price.As in[21],we assume that these“derivatives”are bounded(see Sect.2for the exact deﬁnitions).However,we do not impose uniform bounds over all admissible strate-gies.The price to pay for this relaxation is that we are no longer happy with the mere L2-convergence but price contingent claims using super-replication arguments.We show that a trading strategy that attains the minimal super-replication cost is a per-fect replicating strategy,and its cost of construction contains a liquidity premium,in contrast with the results of[4].A related work on such trading restrictions can be found in Longstaff[21],who suggests a uniform bound on the time derivative of trading strategies to study the optimal portfolios in an illiquid market.More recently,Rogers and Singh[26]studied the Merton problem under liquidity costs where they placed similar restrictions on the trading strategies.Under our admissibility condition,we show in Proposition3.1that strategies with jumps are not optimal so that the super-hedging problem can be restricted to con-tinuous hedging strategies.This feature of our admissibility set is thus in agreement with the conventional wisdom according to which it is better to place consecutive small trades rather than a large one at once in illiquid markets.Our main result,The-orem3.3,proves that the super-replication price V is the unique viscosity solution of the dynamic programming equation−V t−s 2σ24− 2+(V ss+ )+2=0,(1.1)where the function >0is the liquidity index of the market deﬁned in(2.4)below. In fact,for more liquid markets, is larger,with =∞corresponding to the com-plete Black–Scholes ing this equation,it is easy to show that,unless the payoff is afﬁne,the solution to this equation is strictly larger than the Black–Scholes price.Hence there is a liquidity premium.This is proved in Corollary3.4.Moreover, this liquidity premium can be calculated numerically using available methods for the solutions of PDEs of type(1.1).These results are proved by the techniques developed in a series of papers by Soner and Touzi[29–32],Cheridito et al.[7,8],and Cheridito et al.[9].Although the set of admissible strategies that we consider is motivated by tech-nical integrability conditions,our results are supported by a formal description of the corresponding hedging strategy which has a relevantﬁnancial interpretation.As we illustrate in Sect.4,the optimal hedging strategy exhibits an asymmetry between claims with convex and concave payoffs.For derivatives with convex payoff,the hedging strategy is of dynamic Black–Scholes type.However,when the claim to be hedged has a concave payoff,there are two options for the trader:either employ a buy-and-hold strategy at a higher cost of construction but no further liquidity costs, or employ a perfect Black–Scholes-type replicating strategy but expect liquidity costs growing over time.Depending on the market conditions,it might be cheaper to use the buy-and-hold type hedge rather than the replicating strategy when the liquidity cost associated with the latter is expected to be high.In Sect.4we show that this decision should be based on the level of concavity of the value function for the min-imal super-replication price and give a precise level below which it is cheaper to use a buy-and-hold strategy.Options with liquidity cost321 The outline of the paper is as follows.Section2formulates the problem.Section3 presents the main results.Section4describes the formal hedging strategy.Section5ﬁnds the growth condition for the value function,while Sect.6shows the unique-ness of the solution.In the Appendix,we discuss the convexity properties and an illustrative example.2Problem formulationThroughout this paper,weﬁx aﬁnite time horizon T>0,and we consider a one-dimensional Brownian motion W={W(t),0≤t≤T}deﬁned on a complete prob-ability space(Ω,F,P).We denote by F={F(t),0≤t≤T}the smallestﬁltration that contains theﬁltration generated by W and satisﬁes the usual conditions.2.1Theﬁnancial marketTheﬁnancial market consists of two assets,and the objective of the investor is to optimally allocate his wealth between these assets in order to hedge some contingent liability.Theﬁrst asset is nonrisky.Without loss of generality,we normalize its price to unity,which means that this asset is taken as the numéraire.The risky asset is subject to liquidity costs.FollowingÇetin et al.[4],we account for the liquidity costs by modeling the price process of this asset as a function of the exchanged volume.We thus introduce a supply curveSt,S(t),ν,whereν∈R indicates the volume of the transaction,the process S(t)=S(t,S(t),0) is the marginal price process deﬁned by the stochastic differential equationdS(r) S(r)=μr,S(r)dr+σr,S(r)dW(r)(2.1)and some given initial condition S(0),and S:R+×R+×R→R is a smooth func-tion representing the price per share for some given volume of transaction and the marginal price.In addition to the technical conditions imposed in[4]on the supply curve,we assume for each t and s that∂S∂ν(t,s,0)>0.In order to ensure that the stochastic differential equation(2.1)has a unique strong condition,we assume that the coefﬁcient functionsμ,σ:[0,T]×R+→R satisfy the usual local Lipschitz and linear growth conditions.In order to exclude arbitrage opportunities,we assume the existence of an equiva-lent martingale measure P0,i.e.,dS(r) S(r)=σr,S(r)dW0(r),322U.Çetin et al. where W0is a standard Brownian motion under P0,so that the process S is a martin-gale under P0.We shall frequently move the time origin from zero to an arbitrary t∈[0,T],and we then denote by{S t,s(r),r∈[t,T]}the process deﬁned by(2.1)and the initial condition S t,s(t)=s.2.2Trading strategiesA trading strategy is deﬁned by a pair(X,Y),where X(t)denotes the wealth in the bank,and Y(t)is the number of shares held at each time t in the portfolio.For reasons which will become clear later,we restrict the process Y to be of the formY(r)=N−1n=0y n1{r≥τn+1}+rtα(u)du+rtΓ(u)dS t,s(u),(2.2)so that it hasﬁnite quadratic variation.Here,t=τ0<τ1<···is an increasing se-quence of[t,T]-valued F-stopping times,the random variableN:=inf{n∈N:τn=T}indicates the number of jumps,y n is an R d-valued,F(τn)-measurable random vari-able satisfying y n1{τn=T}=0,andαandΓare two F-progressively measurable realprocesses.We shall show in the next section that it is not optimal to have jumps in Y.We continue by deriving the continuous-time dynamics of our state variables.This derivation follows the discrete-time argument of[4].Let t=t0<···<t n=T be a partition of the interval[0,T],and setδψ(t i):=ψ(t i)−ψ(t i−1)for any functionψ. By the self-ﬁnancing condition,it follows thatδX(t i)+δY(t i)St i,S(t i),δY(t i)=0,1≤i≤n.Summing up these equalities,it follows from direct manipulations that X(T)+Y(T)S(T)=X(t)+Y(t)S(t)−ni=1δY(t i)St i,S(t i),δY(t i)+Y(t)S(t)−Y(T)S(T)=X(t)+Y(t)S(t)−ni=1δY(t i)S(t i)+Y(t)S(t)−Y(T)S(T)−ni=1δY(t i)St i,S(t i),δY(t i)−S(t i)Options with liquidity cost323=X(t)+Y(t)S(t)+ni=1Y(t i−1)δS(t i)−ni=1δY(t i)St i,S(t i),δY(t i)−S(t i).(2.3)The continuous-time dynamics of the processZ:=X+Y Sare obtained by taking limits in(2.3)as the time step max{(t i−t i−1),1≤i≤n}of the partition shrinks to zero.The last sum term in(2.3)is the term due to the liq-uidity cost.Under the imposed smoothness assumption onν→S(t,s,ν),it follows thatni=1δY(t i)St i,S(t i),δY(t i)−St i,S(t i),0−→ Ttd[Y,Y]c r4 (r,S(r))+N−1k=0y kSτk,S(τk),y k−S(τk)in probability,where(t,s):=4∂S∂ν(t,s,0)−1.(2.4)In view of the form of the continuous-time process Y in(2.2),this providesZ(r)=Z(t)+rt Y(u)dS(u)−rt14 (r,S(r))Γ(r)2σr,S(r)2S(r)2dr−N−1k=0y kSτk,S(τk),y k−S(τk)1{r<τk+1}.(2.5)In the absence of jumps in the portfolio process,the process Z approaches the classi-cal wealth process in frictionless markets for large .Therefore,we refer to as the liquidity index of the market.In the absence of liquidity costs,the process Z represents the total value of the portfolio of the investor.In the present setting,we assume that the investor is not subject to any liquidity cost at theﬁnal time T.Then,although the process Z has no directﬁnancial interpretation,itsﬁnal value Z(T)is the total value of the investor portfolio at time T.A discussion of initial and terminal liquidity costs is given in Remark3.2.324U.Çetin et al.2.3Admissible trading strategies and the hedging problemThe purpose of the investor is to hedge without risk some given contingent claimG =g S(T ) for some function g :R +→R .In order to formulate the super-hedging problem in the context of our ﬁnancial market with liquidity cost,we need to restrict further the trading strategies as in [32].For B,b ≥0,and for an F -progressively measurable process {H (r),t ≤r ≤T }taking values in R ,we deﬁneH B,b t,s := sup t ≤r ≤T |H (r)|1+|S t,s (r)|B L b (Ω,F ,P ).Throughout the paper,we ﬁx B >0.A trading strategy Y deﬁned by (2.2)is said to be admissible if there is a parameter b >0such thatN ∞<∞,Y B,∞t,s + Γ B,∞t,s + α B,b t,s <∞,and the process Γis of the formΓ(r)=Γ(t)+r t a(u)du + r t ξ(u)dW (u),where a and ξare two real-valued F -progressively measurable processes satisfyinga B,b t,s + ξ B,2t,s <∞.Clearly a larger parameter B implies a larger admissible set.Hence,the parameter B can be viewed as an indicator of market depth.We refer to [8]and [32]for a justiﬁcation of such restrictions.In addition,the already discussed convergence result of the binomial model [19]provides further support for this class of trading strategies.Also,notice that we use the framework of [32],where the restrictions on the drift terms αand a are relaxed compared to [8].This relaxation plays a crucial role in the present paper because,in contrast with our previous work [8],the state variable Z in (2.5)exhibits a jump term.The collection of all admissible trading strategies Y ={Y (r),0≤r ≤T }is de-noted by A t,s .For every Y ∈A t,s ,we denote by Z Y t,z the process deﬁned by (2.5).The purpose of this paper is to solve the super-hedging problemV (t,s):=inf z ∈R :Z Y t,z (T )≥g S t,s (T ) for some Y ∈A t,s .(2.6)Notice that this formulation ignores the liquidity cost both at the time origin t and the ﬁnal time T .As a consequence of Proposition 3.1below,the elimination of the initial liquidity cost does not entail any loss of generality,see Remark 3.2.However,the absence of a liquidity cost at the ﬁnal time is a standing assumption throughout the paper.Options with liquidity cost 325In the subsequent section,we shall prove that we can restrict the portfolios to be continuous,so that the above value function V coincides withV cont (t,s):=inf z ∈R :Z Y t,z (T )≥g S t,s (T ) for some Y ∈A cont t,s ,(2.7)where A cont t,s consists of all continuous portfolio processes in A t,s .3Main resultsWe need the following mild technical conditions.The ﬁrst assumption is needed to ensure that the value function is locally bounded.It imposes thatg is bounded from below and sup s>0g(s)1+s <∞.(3.1)Indeed,the lower bound on g is immediately inherited by V ,and the afﬁne growth condition guarantees the existence of a trivial buy-and-hold strategy which super-hedges the contingent claim g(S(T )),thus producing a locally bounded upper bound for g ,see Proposition 5.1below.We place on the volatility function the standard condition thatσis bounded and Lipschitz-continuous .(3.2)The following condition on the liquidity function is needed for the comparison result of Sect.6:is locally Lipschitz-continuous ,and δ:=inf (t,s):δ≤s ≤δ−1,t ∈[0,T ] >0for every δ>0.(3.3)3.1Optimality of continuous portfoliosIn this subsection,we ﬁrst prove the optimality of continuous portfolio processes.Intuitively,it is clear that in an illiquid market it is better to make consecutive small trades instead of a large one.Then taking this idea to the limit,we formally see that jumps in the portfolio are not optimal.The following result proves this intuition.From the technical viewpoint,let us stress that the relaxation on the processes αand a plays a crucial role for the next result so that our deﬁnition of the set of admissible strategies allows one to preclude jumps from optimal strategies,thus agreeing with economic intuition.Proposition 3.1Assume (3.1),(3.2),and (3.3).Then V cont =V .Proof Fix (t,s)in [0,T )×(0,∞).The inequality V cont (t,s)≥V (t,s)is obvious as A cont t,s ⊂A t,s .To prove the reverse inequality,let z >V (t,s)and Y ∈A t,s be such that Z(T )≥g(S(T ))a.s.We denote by τ1,...,τN the jump times of the portfolio process Y .From the deﬁnition of admissible strategies,recall that N ∞<∞.326U.Çetin et al.Letε>0be given.Weﬁrst start by eliminating theﬁnal jump at timeτN. Notice that Z Y t,z(T)=Z YτN,ZτN(T)≥g(S(T))a.s.Then,with z N:=z+ε,it fol-lows from Lemmas5.2and5.4in[32]that Z Y N t,zN (T)≥g(S(T))a.s.for someY N∈A t,s with Y N=Y on[t,τN)and such that(Y N,ΓN)is continuous on (τN−1,T].Repeating the above procedure backward,we may eliminate all the jumps.Hence,with z0:=z+ε N ∞,there exists Y0∈A contt,s such that Y t,z0,Y0(T)≥g(S(T))a.s.Hence,z0≥V cont(t,s).Sinceε>0and z>V(t,s)are arbitrary,we conclude that V(t,s)≥V cont(t,s).We close this subsection by discussing the initial andﬁnal liquidation costs.Remark3.2The previous result on the continuity of the optimal portfolio also proves that there is no initial liquidity cost.Indeed,suppose that we start with a portfolio value different than the optimal one.Then by shifting the initial wealth by ,we can use Proposition5.1of[30].This shows that we can construct a super-replicating portfolio with an arbitrary initial position in the risky asset as long as the initial wealth in the bank account is larger by .The situation at theﬁnal time is different.At maturity,we are forced to liquidate. This results in a liquidation cost.We chose to ignore this in our analysis.Including this cost will make the liquidity premium even larger.Hence this assumption does not affect the main result of this paper,namely the existence of a liquidity cost.On the other hand,thisﬁnal liquidity cost can be driven to zero if a nonzero amount of time is given for liquidation.The liquidity premium that we prove to exist,however,is due to continuous-time trading.Moreover,this premium cannot be avoided by spreading our trades over time. This is the motivation behind ignoring theﬁnal liquidity cost.3.2The dynamic programming equation characterizationIn this subsection,we prove the viscosity property of the minimal super-replication cost.Let V and V cont be as in(2.6)and(2.7).Theorem3.3Assume(3.1),(3.2),(3.3)and that the payoff function g is continuous. Then V=V cont is the unique continuous viscosity solution of the dynamic program-ming equation−V t+ˆH(t,s,V ss):=supβ≥0−V t−12s2σ2(V ss+β)−s2σ24(V ss+β)2=0(3.4)on[0,T)×(0,∞),satisfying the terminal condition V(T,·)=g and the growth condition−C≤V(t,s)≤C(1+s),(t,s)∈[0,T]×R+,for some constant C>0.(3.5)The proof of this theorem is completed in several steps.The viscosity property of the value function and the terminal data follows from a general result proved in Theorem3.2of the paper[32].The growth condition(3.5)is derived in Sect.5. Finally,the uniqueness result follows from the comparison result of Sect.6.We close this subsection by several observations on the structure of(3.4).First, observe that the dynamic programming equation(3.4)is parabolic,i.e.,nonincreasing in V ss,as all dynamic programming equations should be.Moreover,the differential operator appearing on the left-hand side of(3.4)is the parabolic envelope of theﬁrst guess operator−V t+H(t,s,V ss):=−V t−12s2σ2V ss−s2σ24V2ss.We refer to[7]for more details on the construction of parabolic majorantsˆH of H.Finally,by direct manipulation,we see that the maximizer in the dynamic pro-gramming equation(3.4)is given byˆβ(t,s):= V ss(t,s)+ (t,s) −,(3.6) so that we can rewrite the dynamic programming equation as(1.1).3.3Liquidity premiumLet V BS be the Black–Scholes price of the claim g.Clearly,V≥V BS,and the liquid-ity premium is the difference.Our next result states that the liquidity premium is zero only for afﬁne payoffs.Corollary3.4Assume that the hypotheses of Theorem3.3hold.Then V=V BS if and only if g is an afﬁne function.Hence the liquidity premium is nonzero for all nontrivial claims.Proof By the deﬁnition ofˆH,it is easily seen that−V t−H(t,s,V ss+ˆβ)=0,whereˆβ≥0is given by(3.6).If V=V BS,then V is smooth and is a classical so-lution both of the above equation and the Black–Scholes equation.This immediately implies thatˆβ=V ss=0.Then g is afﬁne.The reverse implication is trivial by veri-fying that afﬁne functions satisfy the PDE of V.4Formal description of an optimal hedging strategyWe now provide a formal description of an optimal hedging strategy for a payoff g(S T)under liquidity costs.An illustrative example is studied in Appendix A.2.The analysis of this section will be restricted to a formal discussion as we shall ignore some admissibility restrictions and regularity conditions.For concreteness,we work in the context of the classical Black–Scholes model,i.e.,σ(t,s)≡σfor some positive constant σ.This would also enable us to com-pare our results with the classical Black–Scholes formula.We also assume that is independent of the t -variable.4.1Usual hedgeWhen the minimal super-replication cost V is a classical solution of (3.4)and if ˆH(t,s,V ss (t,s))=H (t,s,V ss (t,s))for all (t,s),then the usual hedge is replicating.We ﬁrst state and prove this result.Then,in the Appendix,we provide sufﬁcient conditions on .First recall that ˆH(t,s,V ss (t,s))=H (t,s,V ss (t,s))if and only if we have V ss (t,s)≥− (t,s).This condition is equivalent to the convexity ofˆV (t,s):=V (t,s)+ s 1s 1 (t,s )ds ds .Theorem 4.1Let V be the value function .Assume that ˆVdeﬁned above is convex .Then V is smooth and is a classical solution of (3.4).Moreover ,the classical hedge Y (u)=V s (u,S(u))is replicating .Proof We know that V is a viscosity solution of (3.4).Moreover,the convexity of ˆVdeﬁned above implies that the optimizer βin (3.4)is zero and that (3.4)is locally uni-formly elliptic with a convex nonlinearity.Then,one can use the celebrated regularity result of Evans [15]and Krylov and Safanov [22,23]to conclude that V is smooth.Therefore,it is a classical solution of (3.4).Since (3.4)is a parabolic equation in one space dimension,one can also prove this regularity result directly without referring to the deep regularity theory of Evans and Krylov.Indeed,a ﬁxed-point argument using the results and the techniques from the classical textbook of Ladyzhenskaya et al.[24]also yield this regularity.Moreover,V ss (t,s)>− (t,s)∀(t,s),(4.1)and (3.4)holds with ˆH(t,s,V ss (t,s))=H (t,s,V ss (t,s)).Then,by a standard ap-plication of Itôcalculus,we can show that the classical hedge Y (u)=V s (u,S(u))is replicating. In Appendix A.1,we discuss two sufﬁcient conditions for (4.1).4.2Buy-and-hold versus dynamic hedgingIn this subsection,we discuss the general structure of the hedge.An illustrative ex-ample with g(s)=s ∧1will later be discussed in Appendix A.2.To simplify the discussion,we assume that the supply function is of the formS (t,s,ν):=se αsν/4so that (s)=1αs .。

Non-Central Beta Type 3 Distribution

where 0 < u < 1 and the conﬂuent hypergeometric function 1 F1 has the integral representation (Luke [9, Eq. 4.2(1)]),
1 F1 (a; c; z )
=
1 B (a, c − a)
1
ta−1 (1 − t)c−a−1 exp(zt) dt,
It is well known that if X and Y are independent random variables having a standard gamma distribution with shape parameters a and b, respectively, then X/(X + Y ) ∼ B1(a, b), X/Y ∼ B2(a, b) and X/(X + 2Y ) ∼ B3(a, b). The random variable U is said to have a non-central beta type 1 distribution if its p.d.f. is given by NCB1(u; a, b; δ ) = exp (−δ ) ua−1 (1 − u)b−1 1 F1 (a + b; b; δ (1 − u)) , B (a, b) (4)
Non-Central Beta Type 3 Distribution Daya K. Nagar[a],* and Yeison Arley Ramirez-Vanegas[a]
[a]
Instituto de Matem´ aticas, Universidad de Antioquia, Calle 67, No. 53–108, Medell´ ın, Colombia.

T1和E1基础知识

1T线路基础要理解T线路，就不得不对TDM（Time—Division Multiplexing，时分复用）技术有所了解。

在没有TDM技术之前，用户一旦需要从网络服务商那里得到某种端到端的服务就需要专门申请一条线路来部署网络。

很明显,这是不合算的。

因为这种端到端的服务通常是按传输距离计费的,而不是按实际使用的网络带宽来收费的.但采用了TDM技术之后，就没有这么麻烦了.只要用户在以前与对应网络服务商建立了端到端的专用线路,下次在需要其他服务时，就不必另外配置端到端的网络链路了，因为它可以直接复用在原来的专用线路中，如图6—1所示.这其实就是共享线路，即“复用"的意义，也就是T线路的技术所在。

而TDM则可以把复用在一条线路的多条业务链路以时间段为单位轮流分配给不同的业务使用,通常在业务不是很繁忙，或者业务流量不是很大的情况下，对各业务的影响是非常小的，特别是对非实时的业务,如数据查询、文件浏览、数据传输等.它节省了用户大笔的网络接入费用,因此受到了用户的青睐。

T线路是专用线路,用户使用前必须向网络服务提供商（NSP,一般是电信公司）申请。

用户首先从电信局在每个地点之间租用一条专用线路，然后安装管理这些地点间的分组通信流动的交换设备。

它之所以被称为专用网，是因为用户的设备直接控制着每个租用线路地点的通信情况。

与此相反，分组交换技术，例如，帧中继、交换式多兆位数据服务（SMDS）和异步传输模式（ATM），在散列技术的支持下,提供任何地点对任何地点的连接。

这时,一个分组是一个完整的、被编址的数据包，它被转发通过分组交换散列网络上的中继器，直到抵达它的目的地.1．T线路级别划分在T线路中，根据数据速率和信道的多少，可划分为T1、T2、T3这3级，也就是TDM复用级别。

不过在正式介绍以上3种复用级别前，还要对一种被称为“标准数字服务速率”的术语有所了解。

因为以上3种复用级别就是根据这个标准数字服务速率来划分的。

Dialog - Power Management Solutions for Smartphone

S N O I T U L O SFOR SMARTPHONESDialog’s power management solutions are optimized to meet the challenging needs of today’s smartphones. Tight space constraints, thermal challenges and ever changing power requirements are effectively addressed through Dialog’s portfolio of highly integrated, highly efficient and easily configurable PMICs.Dialog also offers innovative, highly efficient and high-density charging solutions that reduce heat dissipation for any rapid charging technology.Development SupportThroughout the customer product development period, Dialog provides quick and expert application support. Dialog’s local field application engineers are always on hand to provide dedicated advice and guidance during the critical design phase.Custom Mixed-Signal Solutions.....................Page 04Power management ICs ...................................Page 06Switched Capacitor DC-DC Converters ....Page 11Smartphone charging ICs ................................Page 13Power conversion solutions ............................Page 15Haptics ......................................................................Page 24ContentsSUNNY38CapacitiveDividersThe industry’s first andhighest efficiency Switch CapConverters with extremelysmall footprint enabling 2S to1S voltage conversions withoutrequiring inductorsCustom Mixed-Signal SolutionsMarket leading CustomPMICs and Mixed-SignalASICs deliver customizedsolutions to optimizeefficiency, size, performanceCustom Mixed-Signal SolutionsDialog Semiconductor, a market leader in custom Power Management ICs (PMICs) and Mixed-Signal ASICs, has a proven track record and long history in its close collaboration with industry leaders to deliver customized, high-performance solutions. The company’s exemplary record has resulted in multi-generational partnerships which have firmly established Dialog as a trusted partner of choice. Integrate to DifferentiateDialog enables many of the most unique and advanced electronics products on the market today. Its broad, mixed-signal portfolio ranges from advanced power conversion topologies, battery chargers, audio solutions, digital and analog interfaces to linear analog and signal conditioning technologies. Dialog’s digital capabilities include embedded computing as well as memory to empower next generation solutions. Advanced packaging technologies co-developed with the company’s packaging experts and vendor partners continue to deliver leading edge solutions in the mixed-signal market. Time-to-MarketDialog is now the leading pure play provider of advanced Power Management ICs. This level of success was achieved through absolute attention to detail. Dialog ensures that all IP is proven to perform as designed. Its accumulated library of leading-edge technologies enables Dialog to execute flawlessly and deliver “First Time Right” solutions.Operational ExcellenceDialog’s long-standing relationships with world-class manufacturing partners for wafer fabrication back-end assembly and test provide access to advanced mixed signal process nodes. Expertise in mixed-signal ASIC design and development from concept to production guarantees the highest levels of quality. Dialog’s unparalleled high-volume production ramp capability (from zero to 80 M units within a quarter) empowers its customers to rapidly deliver new products to market. The internal supply chain team at Dialog has delivered 99%+ on-time-delivery (OTD) while working closely to react to today’s dynamic and volatile market conditions.A representative example of a high-performance Custom ASIC in productionI CAudio• Audio Amps • Audio Codecs • Audio Processors • Integrated Power and AudioSolutionsInterface• USB Phy• Type-CController• I2C/I3C• SPI• UART• Display DriversComputing• CPU/MCU- ARM- 8051- DSP- Custom• Memory- Flash- OTP- MTPPowerManagement • DC/DC Converters- Buck- Boost- Buck-Boost • Hybrid Converters • Switch-Cap Converters • LDOsBatteryChargers• Linear Charging• SwitchmodeCharging• Direct Charging• Current Doublers/Voltage Dividers• Power PathManagementLinear Analog& SignalConditioning• ADC• DAC• Op-Amps• Comparators• References• Sensor Interface• Integrated MotorDriversPower management ICs Highly integrated solutions offer space savingand cost-effective smartphone solutionsThe integration of multiple switching regulators and LDOs meets the diverse power management needs of advanced SOCs and conserves valuable board space. Additionally, these highly integrated solutions deliver higher reliability, reduced complexity and provide layout flexibility.Flexible and configurable for multiple SOC platformsDialog’s comprehensive solutions meet the challenges of powering today’s advanced application processor and modem SOCs with diverse voltage and current needs, multiple channels, power sequencing requirements and the ability to scale to higher power needs.PMIC and sub-PMIC families meet wide-ranging needs in today’s applicationsIn addition to highly integrated PMIC solutions with integrated multiple buck regulators and LDOs, Dialog offers a family of high-current sub-PMICs that satisfy the high-current needs of the latest SOCs. These sub-PMICs offer multi-phase, high-current (up to 20 A) buck regulators in a compact footprint with advanced power management features.System PMIC Product PortfolioSub-PMIC Product PortfolioEasy development with SmartCanvas™ and Power Commander user interfaceThe Dialog SmartCanvas and Power Commander software equips hardware and software developers with a powerful graphical interface for viewing and controlling Dialog system PMICs, sub-PMICs, chargers, andDC/DC converters. The benefits include:Any start-up sequence can be quickly tested in real-timeEasy setup of the fully-programmable sequencer for bucks, LDOs, GPIOs, and system control signalsConfiguration of up to three power domains which are selectable via the sequencer, by GPIOs, or by software Fast debugging using the I 2Cinterface for full visibility of the PMIC statusComplete solutions for smartphone devices:Switched Capacitor DC-DC ConvertersDialog Semiconductor is first in the industry to launch DA9313, an ultra-efficient Buck Converter that does not require the use of inductors. This differentiated technology is leading the way in optimizing next-generation mobile devices.This family of devices - also referred to as Switched Cap Dividers - can offer up to 98% efficiency at high power levels. The combination of “almost loss-less” conversion and high power density make it ideally suited to power the emerging design trends in Smartphones. Major benefits of this unique technology include direct charging of single cell Lithium Ion batteries - enabling usage of standard 3A low-cost USB cables to deliver up to 6A of charging current, 75% reduced routing losses and more than 50% reduction in PCB board area – all within a package height of less than 1mm.The DA9313 – Dialog’s 1st-Gen offering - can provide up to 10A of current in a 10mm2 offering with an extremely flat efficiency curve at a peak efficiency of 98.2% over a wide range of output current loads. It can also operate in a Master-Slave configuration to deliver up to 20A. The DA9313 also offers enable and power-good functions and can be configured to operate in a fixed-frequency mode.The next-generation of this unique technology is in development and will be introduced to key customersduring the 1st half of 2019 and to the broader market in late 2019.Smartphone charging ICsDialog offers a high-efficiency companion charger solution for rapid charging delivering up to 2.5 A charging current, and direct charging solutions that can deliver up to 6 A charging current to the battery using a standard 3 Amp USB cable. Dialog’s highly efficient inductor-less switched capacitor DC/DC converter enables 1S mobile devices to migrate to 2S battery configuration without any changes in the regulated voltage rails.The DA9318 is a high-voltage inductor-less current doubler powering up to 44 W, with integrated protection features and incredible power efficiency of up to 98%, and has an input voltage range of 5.5 V to 10.5 V. The DA9155M is a 2.5 A companion charger with 92% peak efficiency for 1S battery applications, and has aninput voltage range of 4.3 V to 13.5 V for all rapid charging technologies.Eff CurveHighly integrated,configurableand scalablesolutions that are compact and efficient to fully meet the powermanagement needs of today’s smartphones.AC/DC RapidCharge™ controller solutionsAs the leader in AC/DC RapidCharge solutions, Dialog supports more fast-charging protocols than any other supplier, including USB Power Delivery 3.0 (USB PD 3.0); Qualcomm® Quick Charge™ 4+, 3.0 and 2.0 technologies; Samsung Adaptive Fast Charging (AFC); direct charge; and other proprietary OEM protocols.USB Power Delivery 3.0Qualcomm® Quick Charge™ 4+Qualcomm® Quick Charge™ 3.0Qualcomm® Quick Charge™ 2.0Samsung Adaptive Fast ChargingDirect ChargeOther Proprietary OEM ProtocolsQualcomm® Quick Charge™ is a trademark of Qualcomm® Technologies Inc.iW662+iW1796 – low BOM cost 27W Qualcomm ® Quick Charge™ 3.0 adapter solutioniW662+iW1798 – high-power 45W Qualcomm ® Quick Charge™ 3.0 adapter solutioniW657P+iW1799+iW676 – complete system solution for USB Power Delivery 3.0 travel adaptersAC/DC RapidCharge™ adapter solutions(1) DLNK is Dialog’s digital communication from secondary to primary side via an optocoupler.(2) XM-Comm is Dialog’s proprietary digital communication via the main power transformer that eliminates the need for an optocoupler.Qualcomm ® Quick Charge™ is a product of Qualcomm Technologies, Inc.iW1602+iW676 A C/DC high power density travel adapter solution Dialog’s iW1602+iW676 chipset offers an unmatched combination of high efficiency, low standby power and fast dynamic load response DLR(1) enabling smartphone manufacturers to pack more power (higher power density) into smaller travel adapters without incurring thermal issues.iW1602 primary-side controllerPrimAccurate™ primary-side current sensing eliminates secondary-side sense resistorC onfigurable light load operation mode optimizes for low no-load power consumption and fast DLRUp to 30 W outputiW676 secondary-side active voltage position (AVP) controllerBuilt-in synchronous rectifierEnables < 30 mW no-load power consumption and fast DLRLow BOM cost80 mV ripple with single 470 µF output capLow cost slow diodesSOT23 package(1) DLR: Dynamic Load Response - defined as Vout voltage deviation for load step from low to high output current.Ideal for compact, high power density 5 V, 2 A 10 W smartphone adapters/chargersDouble-layer cable protectionDedicated pins for external over-voltage protection with latch (iW1602)SmartDefender TM advanced hiccup technology (iW1602)Robust protection featuresOutput short circuit protectionOutput over-voltage protectionOutput over-current protectionOver-temperature protectionTM21202322AC/DC secondary-side ICsEliminates parallel Schottky diode for lower BOM cost Added benefits of iW67625 V output, optimized for lowest BOM cost in applications up to 12 VOptimized for direct charging applications down to 3 VIncorporates AVP (Active Voltage Positioning) for fast dynamic load responseDigital synchronous rectifiers replace Schottky diode for higher efficiency, ultra-compact power adapters – iW673, iW676Features and BenefitsV OUTGND2524HapticsHaptics technology recreates the sense of touch to the user by applying forces and vibrations in the form of touch feedback in displays, touch surfaces and buttons.The DA728x - High definition, low power and flexible:The DA728x family of High-Definition (HD) Haptic Drivers, combine custom drive sequences, on- and off resonance, at up to 1kHz. The device can drive both ERM and LRA (narrow and wideband) actuators and track resonance up to 300Hz to drive the most complex click/vibration touch effects in a wide range of applications.Saving power when idle:As haptic drivers spend the majority of their time in standby/idle mode, the DA728x family was designed to utilize very low idle current consumption (360nA) to maximize battery life.The DA7282 has an additional standby mode where current consumption drops to 5nA, making it an ideal driver for systems where the highest priority is current consumption when not in use.The DA728x family combines very low idle current consumption with low-latency trigger inputs to minimize system power consumption. Integrated trigger inputs allow haptic operation without waking the application processor, and latency times at sub-1ms give real switch behavior - 10x better than any other solution available today.Simplifying usage in touchscreen and capacitive touch systems:SCLSDAnIRQGPI_() /PWM GPI_1GPI_2External Trigger 1GPI_0262728Hong K ong+86 755 2981 3669China (Shenzhen)+86 21 5424 9058China (Shanghai)****************+852 3769 5200Ko rea+82 2 3469 8200Unit ed Kingdom GermanyThe Netherlands+1 408 845 8500North AmericaJapanTaiwan +31 73 640 8822+81 3 5769 5100Dialog Semiconductor Worldwide Sales Offices -+886 2 80718888+49 7021 8050+44 1793 757 700This publication is issued to provide outline information only, which unless agreed by Dialog Semiconductor may not be used, applied, or reproduced for any purpose or be regarded as a representation relating to products. All use of Dialog Semiconductor products, software and applications referred to in this document are subject to Dialog Semiconductor’s Standard Terms and Conditions of Sale, available on the company website () unless otherwise stated.Dialog and the Dialog logo are trademarks of Dialog Semiconductor plc or its subsidiaries. All other product or service names are the property of their respective owners. © Dialog Semiconductor 2020. All rights reserved.。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

2013年11月份规模以上工业增加值增长10.0% 来源：国家统计局发布时间：2013-12-10 13:30
2013年11月份，规模以上工业增加值同比实际增长10.0 %（以下增加值增速均为扣除价格因素的实际增长率），比10月份回落0.3个百分点；从环比看，11月份比上月增长0.76%。

1-11月，规模以上工业增加值同比增长9.7%。

分经济类型看，11月份，国有及国有控股企业增加值同比增长9.1%，集体企业增长2.4%，股份制企业增长11.1%，外商及港澳台商投资企业增长9.5%。

分三大门类看，11月份，采矿业增加值同比增长5.6%，制造业增长
11.0%，电力、燃气和水的生产和供应业增长6.9%。

分行业看，11月份，41个大类行业中有39个行业增加值保持同比增长。

其中，纺织业增长8.2%，化学原料和化学制品制造业增长12.4%，非金属矿物制品业增长11.3%，黑色金属冶炼和压延加工业增长7.8%，通用设备制造业增长9.9%，汽车制造业增长20.3%，铁路、船舶、航空航天和其他运输设备制造业增长10.3%，电气机械和器材制造业增长12.8%，计算机、通信和其他电子设备制造业增长11.3%，电力、热力的生产和供应业增长6.1%。

分地区看，11月份，东部地区增加值同比增长9.2%，中部地区增长
11.6%，西部地区增长10.0%。

分产品看，11月份，464种产品中有307种产品同比增长。

其中，发电量4392亿千瓦时，同比增长6.8%；钢材9032万吨，增长10.0%；水泥22372万吨，增长10.0%；十种有色金属365万吨，增长13.7%；乙烯150万吨，增长15.4%；汽车228.4万辆，增长25.6%；轿车127.1万辆，增长19.9%；原油加工量4017万吨，下降0.6%。

11月份，工业企业产品销售率为97.6 %，比上年同期下降0.4个百分点。

工业企业实现出口交货值10383亿元，同比名义增长5.8%。

2013年11月份规模以上工业生产主要数据
11月1-11月
绝对量同比增长
（%）
绝对量
同比增长
（%）
一、规模以上工业增加值…10.0 …9.7 分三大门类
采矿业… 5.6 … 6.4 制造业…11.0 …10.5 电力、燃气和水的生产和供应
业
… 6.9 … 6.9 分经济类型
国有及国有控股企业…9.1 … 6.8 集体企业… 2.4 … 4.4 股份制企业…11.1 …11.0 外商及港澳台商投资企业…9.5 …8.2 主要行业增加值
纺织业…8.2 …8.9 化学原料和化学制品制造业…12.4 …12.1 非金属矿物制品业…11.3 …11.7 黑色金属冶炼和压延加工业…7.8 …10.1 通用设备制造业…9.9 …9.3 汽车制造业…20.3 …14.3 铁路、船舶、航空航天和其他
运输设备制造业
…10.3 … 4.3 电气机械和器材制造业…12.8 …10.9 计算机、通信和其他电子设备
制造业
…11.3 …11.2 电力、热力生产和供应业… 6.1 … 6.3 主要产品产量
发电量（亿千瓦时）4392 6.8 47413 7.0 生铁（万吨）5290 0.6 65050 5.9 粗钢（万吨）6088 4.2 71286 7.8 钢材（万吨）9032 10.0 97878 11.5 水泥（万吨）22372 10.0 220088 9.2 原油加工量（万吨）4017 -0.6 43645 3.6 十种有色金属（万吨）365 13.7 3691 10.5 焦炭（万吨）3940 7.2 43595 8.1 硫酸(折100%)（万吨）662 7.6 7358 5.5 烧碱(折100%)（万吨）248 10.7 2603 5.9
化学纤维（万吨）370 8.4 3764 7.6 平板玻璃（万重量箱）6367 12.4 71012 11.6 乙烯（万吨）150 15.4 1475 8.4 微型计算机设备（万台）4121 9.7 31346 7.9 集成电路（亿块）77 11.4 793 10.0 汽车（万辆）228.4 25.6 2161.9 18.1 其中：轿车（万辆）127.1 19.9 1207.6 16.6
产品销售率（%）97.6-0.4(百分点)97.7-0.1(百分
点)
出口交货值（亿元）10383 5.8 102533 4.6
pic:W020131210548411748363_r75.gif
附注
1、指标解释
工业增加值增长速度:即工业增长速度，是用来反映一定时期工业生产物量增减变动程度的指标。

利用该指标，可以判断短期工业经济的运行走势，判断
经济的景气程度，也是制定和调整经济政策，实施宏观调控的重要参考和依
据。

产品销售率：是销售产值和工业总产值的比率，用来反映工业产品的产销衔接情况。

出口交货值：是指工业企业交给外贸部门或自营（委托）出口（包括销往香港、澳门、台湾），用外汇价格结算的产品价值，以及外商来样、来料加工、来件装配和补偿贸易等生产的产品价值。

在计算出口交货值时，要把外汇价格按交易时的汇率折成人民币计算。

日均产品产量：是以当月公布的规模以上工业企业总产量除以该月日历天数计算得到。

产品产量同比增长速度：由于规模以上工业企业范围每年会发生一些变化，按国家统计制度规定，企业在报送当年月度数据时，需同时报送上年同期数。

因此，产品产量同比增长速度是以本期公布的规模以上工业企业总产量除以本期同时报送的同期数据计算得到。

2、统计范围
从2011年起，规模以上工业企业起点标准由原来的年主营业务收入500万元提高到年主营业务收入2000万元。

3、调查方法
规模以上工业企业工业生产报表按月进行全面调查（1月份数据免报）。

4、东、中、西部地区划分
东部地区包括北京、天津、河北、辽宁、上海、江苏、浙江、福建、山东、广东、海南11个省（市）；中部地区包括山西、吉林、黑龙江、安徽、江西、河南、湖北、湖南8个省；西部地区包括内蒙古、广西、重庆、四川、贵州、云南、西藏、陕西、甘肃、青海、宁夏、新疆12个省（市、自治区）。

5、行业分类标准
2012年起，国家统计局执行新的国民经济行业分类标准（GB/T 4754-2011），原来的工业行业大类由39个调整为41个，具体请参见
/tjbz。

6、环比数据修订
根据季节调整模型自动修正结果，对2012年11月至2013年10月份规模以上工业增加值环比增速进行修订。

修订结果及2013年11月份环比数据如下：
月份环比增速（%）
2012年11月0.86 12月0.84
2013年1月0.62 2月0.83 3月0.71 4月0.92 5月0.69 6月0.74 7月0.88 8月0.92 9月0.71 10月0.85 11月0.76。