Kinematic Analysis by Analytical(解析的)

合集下载

机械原理英文词汇

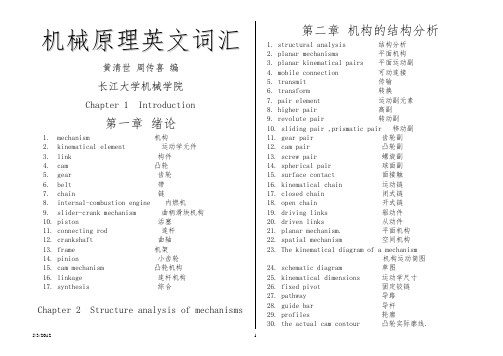
机械原理英文词汇黄清世周传喜编长江大学机械学院Chapter 1 Introduction第一章绪论1.mechanism 机构2.kinematical element 运动学元件3.link 构件4.cam 凸轮5.gear 齿轮6.belt 带7.chain 链8.internal-combustion engine 内燃机9.slider-crank mechanism 曲柄滑块机构10.piston 活塞11.connecting rod 连杆12.crankshaft 曲轴13.frame 机架14.pinion 小齿轮15.cam mechanism 凸轮机构16.linkage 连杆机构17.synthesis 综合Chapter 2 Structure analysis of mechanisms第二章机构的结构分析1. structural analysis 结构分析2. planar mechanisms 平面机构3. planar kinematical pairs 平面运动副4. mobile connection 可动连接5. transmit 传输6. transform 转换7. pair element 运动副元素8. higher pair 高副9. revolute pair 转动副10. sliding pair ,prismatic pair 移动副11. gear pair 齿轮副12. cam pair 凸轮副13. screw pair 螺旋副14. spherical pair 球面副15. surface contact 面接触16. kinematical chain 运动链17. closed chain 闭式链18. open chain 开式链19. driving links 驱动件20. driven links 从动件21. planar mechanism. 平面机构22. spatial mechanism 空间机构23. The kinematical diagram of a mechanism机构运动简图24. schematic diagram 草图25. kinematical dimensions 运动学尺寸26. fixed pivot 固定铰链27. pathway 导路28. guide bar 导杆29. profiles 轮廓30. the actual cam contour 凸轮实际廓线.31. polygon 多边形32. route of transmission 传递路线33. structural block diagram 结构框图34. Degree of Freedom (DOF) 自由度35. constraints 约束36. common normal 公法线37. compound hinge 复合铰链38. gear-linkage mechanism 齿轮连杆机构40. passive DOF 局部自由度41. redundant constraint 虚约束42. The composition principle and structural analysis组成原理与结构分析43. the basic mechanism 基本机构44. Assur groups 阿苏尔杆组45. inner pair 内副46. outer pairs 外副.47. composition principle of mechanism 机构组成原理48. kinematical determination 运动确定性Chapter 3 kinematic analysis of mechanicsms 第三章机构的运动分析1. velocity 速度2. acceleration 加速度3. parameter 参数3. graphical method 图解法4. analytical method 解析法5. experimental method 实验法6. instant center 瞬心7. classification of instant centers 瞬心的分类8. absolute instantaneous center 绝对瞬心9. relative instantaneous center 相对瞬心10. the method of instantaneous center 瞬心法11. the Aronhold-Kennedy theorem阿朗浩尔特-肯尼迪定理(即三心定理)12. the four-bar linkage 四杆机构13. inversion of the slider-crank导杆机构(曲柄滑块机构的倒置机构) 14. complex mechanism 复杂机构，多杆机构Chapter 5 Efficiency and Self-lock ofMachines第五章机械效率和自锁1.pure-slide pair 移动副2.helical pair 螺旋副3.Coulomb’s law 库仑定律4.coefficient of friction 摩擦系数5.rest friction 静摩擦6.kinetic friction 动摩擦7.vee-slot V型槽8.equivalent coefficient of friction 当量摩擦系数9.frictional angle 摩擦角10.equivalent frictional angle 当量摩擦角11.lead angle 导程角12.moment of couple 力偶矩13.clearance 间隙14.frictional circle 摩擦圆15.thrust bearing 推力轴承16.drive work 驱动功17.effective work 有效功18.lost work 损耗功19.ideal machine 理想机械20.serial structure 串联结构21.parallel structure 并联结构22.parallel-serial structure 混联结构23.jack 千斤顶24.self-lock 自锁Chapter 6 Balancing of Machinery第六章机械的平衡1. Vibration 振动2. Frequency 频率3. Resonant 共振4. Amplitudes 振幅5. Balancing of rotors 转子6. Rigid rotors 刚性转子7. Flexible rotors 柔性转子8. Balancing of mechanisms 机构的平衡9. Disk-like rotor 盘状转子10.Non-disk rigid rotor 非盘状转子11.the shaking force 振动力12.the shaking moment 振动力矩13.Balancing of Disk-like Rotors 盘状转子的平衡14.static imbalance 静不平衡15.static balancing machine 静平衡机16.the mass-radius product 质径积17.dynamically unbalanced 动不平衡18.balance planes 平衡基面19.Dynamic balancing machine 动平衡机20.Unbalancing Allowance 许用不平衡量Chapter 7 Motion of Mechanical Systemsand Its Regulation第七章机械系统的运转及其调节1. Periodic speed fluctuation 周期性波动2. punching machine 冲床3. Motion Equation of a Mechanical System机械系统的运动方程4. General Expression of the Equation of Motion运动方程的一般表达式5. the kinetic energy 动能6. the moment of inertia 转动惯量7. Dynamically Equivalent Model of a Mechanical System等效动力学模型8. the equivalent moment of inertia 等效转动惯量9. the equivalent moment of force 等效力矩10.the equivalent link 等效构件11.Pump 泵12.Blower 鼓风机13.Flywheel 飞轮Chapter 8 Planar Linkage Mechanicsms第八章平面连杆机构1.four-bar linkage 四杆机构2.crank-rocker mechanism 曲柄摇杆机构3.double-crank mechanism 双曲柄机构4.double-rocker mechanism 双摇杆机构5.Crashof’s criterion 格拉索夫判据6.Condition for having a crank 有曲柄的条件7.slider-crank mechanism 曲柄滑块机构8.offset distance 偏距9.offset slider-crank mechanism 偏置曲柄滑块机构10.in-line slider-crank mechanism 对心曲柄滑块机构11.rotating guide-bar mechanism. 转动导杆机构12.oscillating guide-bar mechanism 摆动导杆机构13.double rotating block mechanism 双转块机构14.crank and oscillating block mechanism 曲柄摇块机构15.variations 变异16.inversions 倒置17.transmission angle 传动角18.dead point 死点19.imbalance angle 极位夹角20.time ratio 行程速比系数21.quick-return mechanism 急回机构22.pressure angle 压力角23.toggle positions 肘节位置24.oldham coupling 联轴器25.flywheel 飞轮26.clamping device 夹具27.dimensional synthesis 尺度综合28.function generation 函数发生器29.body guidance 刚体导引30.path generation 轨迹发生器Chapter 9 Cam Mechanisms第九章凸轮机构1. contour 轮廓2. Follower 从动件3. Plate cam(or disc cam) 盘形凸轮4. Translating cam 移动凸轮5. Three-dimensional cam 空间凸轮6. cylindrical cam 圆柱凸轮7. Translating follower 直动从动件8. Oscillating follower 摆动从动件9. Camshaft 凸轮轴10.in-line translating follower 对心直动从动件11.offset translating follower 偏置直动从动件12.Knife-edge follower 尖底从动件13.Roller follower 滚子从动件14.Flat-faced follower 平底从动件15.Force-closed cam mechanism 力封闭凸轮机构16.Form-closed cam mechanism 形封闭凸轮机构17.Lift 行程18.cam angle for rise 推程角19.cam angle for outer dwell 远休止角20.cam angle for return 回程角21.cam angle for inner dwell 近休止角22.the quasi-velocity 类速度23.the quasi-acceleration 类加速度24.Constant Velocity Motion Curve 等速运动规律25.rigid impulse 刚性冲击26.Constant Acceleration and Deceleration Motion Curve等加速等减速运动规律27.soft impulse 柔性冲击29.Cosine Acceleration Motion Curve (Simple Harmonic Motion Curve) 余弦加速度运动规律（简谐运动规律）30.Sine Acceleration Motion Curve (Cycloid Motion Curve)正弦加速度运动规律（摆线运动规律）31.3-4-5 Polynomial Motion Curve 3-4-5多项式运动规律bined Motion Curves 组合运动规律33.the cam contour 实际廓线34.the pitch curve 理论廓线35.prime circle 基圆36.the common normal 公法线37.positive offset 正偏置38.negative offset 负偏置39.outer envelope 外包络线40.inner envelope 内包络线41.The locus of the centre of the milling cutter铣刀中心轨迹42.Pressure Angle 压力角43.acute angle 锐角44.the normal 法线45.The allowable pressure angle 许用压力角46.Radius of Curvature 曲率半径47.Cusp 尖点48.Undercutting 根切49.The angular lift 角行程50.interference 干涉Chapter 10 Gear Mechanisms第十章齿轮机构1. constant transmission ratio 定传动比2. planar gear mechanisms 平面齿轮机构3. spatial gear mechanisms 空间齿轮机构4. external gear pair 外齿轮副5. internal gear pair 内齿轮副6. rack and pinion 齿条和齿轮7. spur gear 直齿轮8. helical gear 斜齿轮9. double helical gear 人字齿轮10.spur rack 直齿条11.helical rack 斜齿条12.bevel gear mechanism 圆锥齿轮机构13.crossed helical gears mechanism 螺旋齿轮机构14.worm and worm wheel mechanism 蜗杆蜗轮机构15.Fundamentals of Engagement of Tooth Profiles齿廓啮合基本定律16.the pitch point 节点17.the pitch circle 节圆18.conjugate profiles 共轭齿廓19.transmission ratio 传动比20.involute gear 渐开线齿轮21.the radius of base circle 基圆半径22.generating line 发生线23.unfolding angle 展角24.table of involute function 渐开线函数表25.gearing 啮合26.standard involute spur gears 标准渐开线直齿轮27.the facewidth 齿宽28.addendum circle (or tip circle) 齿顶圆29.dedendum circle (or root circle) 齿根圆30.arbitrary circle 任意圆31.the tooth space 齿槽32.the spacewidth 齿槽宽33.the pitch 齿距，周节34.the reference circle 分度圆35.module 模数36.addendum 齿顶高37.dedendum 齿根高38.tooth depth 齿全高39.the coefficient of addendum 齿顶高系数40.the coefficient of bottom clearance 顶隙系数41.bottom clearance 顶隙42.the normal tooth 正常齿43.the shorter tooth 短齿44.base pitch 基圆齿距，基节45.normal pitch 法向齿距，法节46.conjugated point 共轭点47.proper meshing conditions 正确啮合条件48.working pressure angle 啮合角49.the backlash 齿侧间隙50.the bottom clearance 顶隙51.the reference centre distance 标准中心距52.contact ratio 重合度53.the actual working profile 实际工作齿廓54.the actual line of action 实际啮合线55.manufacturing methods of involute profiles渐开线齿廓的加工方法56.form cutting 仿形法57.generating cutting 展成法或范成法58.disk milling cutter 盘形铣刀59.end milling cutter 指状铣刀60.broach 拉刀ling machines. 铣床62.rack-shaped shaper cutter 齿条插刀63.shaping 插齿64.hobbing 滚齿65.rack-shaped cutter 齿条型刀具the 车床67.cutter Interference 根切68.corrected gears 变位齿轮69.the modification coefficient 变位系数70.positively modified 正变位71.negatively modified 负变位72.the gearing equation without backlash 无侧隙啮合方程73.involute helicoids 渐开线螺旋面74.the transverse plane 端面75.the normal plane 法面78.the transverse contact ratio 端面重合度79.the overlap ratio 轴向重合度80.the virtual gear 当量齿轮81.the virtual number of teeth 当量齿数82.axial thrust 轴向推力83.worm gearing 蜗杆传动84.righthanded 右旋85.lefthanded 左旋86.ZA-worm 阿基米德蜗杆87.involute helicoid worms ----ZI-worm 渐开线蜗杆88.arc-contact worms -----ZC-worm 圆弧齿蜗杆89.enveloping worm 包络蜗杆90.The number of threads 头数91.bevel gears 圆锥齿轮92.back cone 背锥93.virtual gear 当量齿轮94.the reference cone 分度圆锥95.sector gear 扇形齿轮96.the outer cone distance 外锥距97.the reference cone angle 分度圆锥角98.The apexes 锥顶99.The dedendum angle 齿根角100.dedendum cone angle 齿根圆锥角Chapter 11 Gear Trains第十一章轮系1. gear train with fixed axes 定轴轮系2. epicyclical gear train 周转轮系3. elementary epicyclical gear trains 基本周转轮系4. combined gear trains 复合轮系5. planet gear 行星轮6. planet carrier 行星架,系杆,转臂7. sun gears 太阳轮,中心轮8. differential gear train 差动轮系9. the train ratio of a gear train 轮系传动比10.idle wheels 惰轮11.converted gear train 转化轮系12.the efficiency of the gear train 轮系效率13.branching transmission 分路传动14.the brake 刹车片15.the clutch 离合器16.negative mechanism 负号机构17.positive mechanism 正号机构18.train ratio condition 传动比条件19.concentric condition 同心条件20.assembly condition 装配条件21.planetary reducer with small tooth difference少齿差行星减速器22.cycloidal-pin wheel planetary gearing摆线针轮行星传动23.harmonic drive gearing 谐波传动Chapter 12 Other Common Mechanisms 第十二章其它常用机构1. ratchet mechanism 棘轮机构2. pawl 棘爪3. intermittent motion 间歇运动4. geneva mechanism 槽轮机构5. external geneva mechanism 外槽轮机构6. internal geneva mechanism 内槽轮机构7. geneva rack mechanism 齿条槽轮机构8. spherical geneva mechanisms 球面槽轮机构9. the ratio k between motion time and dwell time运动与停歇时间比k10.Cam-Type Index Mechanisms 凸轮式间歇运动机构11.Cylindrical Cam Index Mechanisms圆柱凸轮式间歇运动机构12.Universal Joints 万向联轴节13.The Single Universal Joint 单万向联轴节14.The Double Universal Joint 双万向联轴节15.Screw Mechanisms 螺旋机构16.single-threadscrew mechanisms 单螺旋机构17.Double-thread screw mechanisms 复式螺旋机构18.Index cam mechanism 分度凸轮机构19.Geared linkages 齿轮连杆机构Chapter 13 Creative Design of Mechanism Systems 第十三章机械系统的创新设计1. prototype machine 样机2. Working cycle diagrams 工作循环图3. reference link 定标件4. Circular working cycle diagram 圆工作循环图5. Rectilinear working cycle diagram 矩形工作循环图6. Rectangular coordinate working cycle diagram直角坐标式工作循环图the coefficient travel speed variation K 行程速比系数crank acute angle (imbalance angle) θ极位夹角differential gear train 差动轮系planetary gear train 行星轮系magnitude and direction (大小和方向)compound hinge 复合铰链passive DOF 局部自由度redundant constraint 虚约束offset cam mechanism 偏置凸轮机构pressure angle 压力角base circle 基圆instant centre 瞬心transmission angle 传动角。

机械英语

Chapter 3 Kinematic Analysis of Mechanisms3.1 Tasks and Methods of Kinematic AnalysisThe tasks of kinematic analysis are to find angular positions, angular velocities and angular accelerations of driven links and/or positions, linear velocities and linear accelerations of points on driven links, according to input parameters of driving link(s) and the dimensions of all links.In order to determine whether or not all links will interfere with each other, or to determine the stroke of a driven link, or to find the locus of a point, we must analyse positions of the links and/or the points of interest. Position analysis is also the first step in velocity and acceleration analysis.In order to calculate the stored kinetic energy by formula E=mV 2/2 or E=J ω2/2, or to determine the power P of a motor by formula P=F*V , we carry out the velocity analysis, which is also a step on the way to the determination of the acceleration.Designers must ensure that the stresses in the materials of the proposed machine are kept well below their allowable levels. To calculate the stresses, we need to know the static and dynamic forces on the links. To calculate the dynamic forces, we need to know the acceleration because the inertia forces are proportional to acceleration.Kinematic analysis of mechanisms can be carried out by graphical or analytical or experimental methods. By geometric drawing, the positions of all links in a grade II linkage can be determined easily according to the assembly order of Assur groups, step by step. In this chapter, we will introduce one of the graphical methods, named the instant centres method, for velocity analysis. The analytical method has many advantages over graphical methods. In this chapter, we will put the stress on the analytical method.3.2.1 Definition of the Instant CentreShown in Fig.3-1 are two bodies 1 coincident points, e.g. P 1 and P 2necessary, so that the point P is "in" both absolute velocities of which are the magnitude and direction, i.e. V V P P 12=. At this instant,Fig.3-1there is no relative velocity between this pair of coincident points, i.e.V VP P P P1221==0. Thus, at this instant, either link will have pure rotation relative to the other link about the point. This pair of coincident points with the same velocities is defined as the instantaneous centre of relative rotation, or more briefly the instant centre, denoted as P12or P21. If one of links is the frame, the instant centre is called an absolute instant centre, otherwise, a relative instant centre. The absolute instant centre is the zero-velocity point on a moving link, but its acceleration may not be zero.At the position shown in Fig.3-1, the two links rotate relative to each other about the instant centre P12. So any other pair of coincident points, e.g. A1 and A2, will have relative velocities, i.e. V A1A2 and V A2A1. The directions of V A1A2 and V A2A1 are perpendicular to PA. Therefore, if the direction of the relative velocity of a pair of coincident points, e.g. A1 and A2, is known, then the instant centre must lie somewhere on the normal to the relative velocity passing through the coincident point A.3.2.2 Number of Instant Centres of a MechanismEach pair of links i and j has an instant centre and P ij is identical to P ji. Thus the number N of instant centres of a mechanism with K links isNK K=-*()12Note: The frame is included in the number K.3.2.3 Location of the Instant Centre of Two Links Connected by a Kinematic Pair (1) Revolute pairIf two links 1 and 2 are connected by a revolute pair, as shown in Fig.3-2(a), the centre of the revolute pair is obviously the instant centre P12 or P21.(2) Pure-rolling pairThe pure-rolling pair is a special case of a higher pair, as shown in Fig.3-2(b). There is no slipping between the two contacting points A1and A2, i.e. V A1A2=V A2A1=0. Thus the point of contact A is the instant centre P12or P21. Kinematically, the transmission between a pair of gears is equivalent to rolling without slipping between a pair of circles. So the contact point of the two pitch circles of the gears 1 and 2 is the instant centre P12 for the gears 1 and 2, as shown in Fig.3-2(c).(3) Sliding pairAs can be seen in Fig.3-2(d), relative translation is equivalent to relative rotation about a point located at infinity in either direction perpendicular to the guide-way. Therefore, the instant centre of the two links connected by a sliding pair lies at infinity in either direction perpendicular to the guide-way.The instant centres mentioned so far are called observable instant centres and should be located and labeled before any others are found.(4) Higher pair (rolling & sliding pair)Shown in Fig.3-2(e) are two links 1 and 2 connected by a higher pair. Their contact point is point A. The direction of relative velocities, V A1A2and V A2A1, between A1 and A2 must be along the common tangent. Otherwise there will be a relative velocity component along the common normal n-n which will make the. According to the theorem of three centres, the three instant centres P12 , P13 and P23 must lie on a straight line. This theorem can be proved as follows.Suppose that the positions of P12 and P13 are known, as shown in Fig.3-3. Let us consider any point, e.g. point C, outside the line P12P13. Since P12(A) is the instant centre of the links 1 and 2, the link 2 rotates relative to the link 1 about the point A. So V C2C1⊥AC. Similarly, V C3C1⊥BC. Since V C2=V C1+V C2C1, thenV C2C1=V C2-V C1. Similarly, V C3C1=V C3-V C1. Obviously, for any point Coutside the line P12P13, the directions of the vectors V C2C1 and V C3C1 are not thesame, i.e. VC2C1 ≠V C3C1.(V C2-V C1) ≠(V C3-V C1) from which obtains C2≠C3. Hence, according to be the instant centre P 23 between the links 2 and3. In other words, any point outside the straightline P 12P 13 cannot be the instant centre P 23. Thus the theorem of three centres is derived: the three instant centres of any three independent links in general plane motion must lie on a common straight line.3.2.5 Applications of Instant CentresExample 3-1For the four-bar mechanism shown in Fig.3-4, the angular velocity ω1 of crank 1 is given. For the position shown,(1) locate all instant centres for the mechanism,(2) find the ratio ω3 /ω1 of the angular velocity of link 3 to that of link 1,(3) find the velocity V F of point F on link 2.Solution:(1)There are sixcentres in this four-bar mechanism. In order to should first try to locate observable instant centres. There are four instant centres (P 14, P 12, P 23 and P 34) and two unobservable instantcentres (P 13 and P 24) in this mechanism. According to the theorem of three centres, P 13 will lie not only on the line P 14P 34, but also on the line P 12P 23. Since P 23 is at infinity perpendicular to the guiding bar 2, line P 12P 23 passes through the point P 12 Fig.3-3 Fig.3-4and is perpendicular to BF. Hence the intersection E of the lines P14P34 and P12P23 is the instant centre P13.Similarly, line P23P34 passes through the point P34 and is perpendicular to BF, the intersection G of the lines P12P14 and P34P23 is the instant centre P24. Thus it can be seen that it is usual to apply the theorem of three centres twice to determine two lines, the intersection of which will be the unobservable instant centre. Instant centres P14, P34and P24are absolute instant centres, while the others are relative instant centres.(2) In order to find ω3 for the given ω1, we should take advantage of the frame 4. Their three instant centres(P34, P13, P14) lie on a common straight line. The moving links 1 and 3 rotate relative to the frame 4 about the absolute instant centres P14(A) and P34(D) respectively. In link 1, V E1=ω1*L AE. In link 3, V E3=ω3*L DE. (Extend the two links, if necessary, so that the point E is "in" both links.) Since the point E is the instant centre P13between the links 1 and 3, V E1=V E3.Therefore, ω1*L AE=ω3*L DE from which i31=ω3/ω1= L AE/L DE =P14P13/P34P13. The lengths of L AE and L DE are measured directly from the kinematic diagram of the mechanism. The direction of ω3 is counter-clockwise at this instant.From above, it is shown that the ratio ωi /ωj of angular velocities between any two moving links i and j is equal to the inverse ratio of the two distances between the relative instant centre P i j and two absolute instant centres P f i and P f j , that is,ωωijfj ijfi ijP PP P=----------------------------------------------------------------------(3-1)where the subscript f represents the frame! If the relative instant centre P ij lies between the two absolute instant centres P fi and P fj, then the directions of ωi and ωj are different. Otherwise, the directions of ωi and ωj are the same.(3) Since the links 2 and 3 are connected by a sliding pair, they cannot rotate relative to each other. Thus, ω2 =ω3 =ω1*L AE/L ED. Since P24 is the absolute instant centre, the link 2 rotates (relative to the frame 4) about the point P24(G) at this instant. Therefore, V F=ω2*L GF. Its direction is perpendicular to GF, as shown in Fig.3-4. Note: Although the velocity of the point P24(G) is zero, its acceleration is not zero.Example 3-2In the cam mechanism with translating roller follower shown in Fig.3-5, the cam is a circular disk. Supposing that the angular velocity ω1 of the cam is known,the velocity V 2the position shown.Solution: As mentioned in passive DOF. The velocity of change if the roller is welded normal n-n through the point of contact C. Accordingto the theorem of three centres, P 12 must lie on the straight line connecting P 13 and P 23. Since the links 2 and 3 are connected by a sliding pair and their instant centre P 23 is at infinity perpendicular to the guide way, the line P 13P 23 passes through P 13 and is perpendicular to the guide way. Thus the intersection B of the common normal n-n and the line P 13P 23 is the instant centre P 12 and V B1 =V B2. Note: Neither the centre O of the circle nor the contact point C is the instant centre P 12. On the cam 1, V B1 =ω1*L AB . Since the follower 2 is translating, all points on the follower 2 have the same velocity 2. So V 2 =V B2 =V B1 =ω1*L AB .Example 3-3In Fig.3-6, gear 3 rolls onrack 4 without slipping. velocity 1 of slider 1 is velocity V D is to be found.Solution: In order to find the point on the gear 3, angular velocity ω3 of the gear 3 should be found first. As mentioned before, in order to find ω3 of the gear 3 for the given velocity V 1 of the slider 1, we always take advantage of the Fig.3-5 Fig.3-6frame 4. Thus we should try to locate the three instant centres, P34, P14and P13, between the three links 1, 3 and the frame 4. The gear 3 rolls on the fixed rack 4 without slipping. So the contact point C is their instant centre P34. P14 lies at infinity perpendicular to AB(not AC!). P13 must lie on both lines P14P34 and P23P12. So the intersection E of the lines P14P34and P23P12is the instant centre P13between the links 1 and 3. Therefore V E3 =V E1. Since the slider 1 is translating, V1 =V E1 =V E3=ω3*L CE. Thus ω3 =V1/L CE from which V D =ω3*L CD =V1*L CD /L CE. The direction is as shown in Fig.3-6.3.2.6 Advantages and Disadvantages of the Method of Instant CentresThe method of instant centres offers an excellent tool in the velocity analysis of simple mechanisms. However, in a complex mechanism, some instant centres may be difficult to find. In some cases they will lie off the paper. Lastly, it should be pointed out that an instant centre, in general, changes its location on both links during motion. The acceleration of the instant centre is not zero(except for fixed pivots). Therefore, the instant centre method cannot be used in acceleration analysis.3.3 Kinematic Analysis by Analytical MethodsIn graphical methods, none of the information obtained for the first position of the mechanism will be applicable to the second position or to any others. The kinematic diagram of the mechanism must be redrawn for each position of the driver. This is very tedious if a mechanism is to be analyzed for a complete cycle. Furthermore, the accuracy of the graphical solution is limited.In contrast, once the analytical solution is derived using an analytical method, it can be evaluated on a computer for different dimensions and/or at different positions with very little effort. The accuracy of the solution far surpasses that required for mechanical design problems. Thus, in this chapter we will put stress on the analytical method. Graphical methods can be used if necessary as a check on the analytical solutions.There exist many kinds of analytical methods for kinematic analysis of linkages. The kinematic analysis of a multi-bar linkage mechanism seems to be a hard task at first sight. However, it becomes easier if the Assur-group method is used. As mentioned in Sec.2.5, most linkage mechanisms are built up by adding one or more commonly used Assur groups to the basic mechanism. Since the DOF of an Assur group is zero, Assur groups have kinematic determination. That is, the motions of all links in an Assur group can be determined so long as the motions of all outer pairs are known. Taking this fact into account, one can set up subroutinesin advance for some commonly used Assur groups. Then the kinematic analysis of a multi-bar linkage mechanism is reduced to two simple steps: first, dividing the mechanism into Assur groups and secondly, calling the corresponding subroutine for each Assur group according to the type and the assembly order of the Assur group. This method is called the Assur-group method for kinematic analysis.In the next sections, we will set up some commonly used kinematic analysis subroutines before analyzing a six-bar linkage mechanism.3.3.1 The LINK Subroutineand acceleration of a point A (i.e. X A, Y A, (V A)X, (V A)Y(a A)Yacceleration of link AB(i.e. θ, ω, ε) and the length (L ABlink AB are known, as shown in Fig.3-7. The Xcomponents of position, velocity and acceleration of point B (i.e.Fig.3-7X B, Y B, (V B)X, (V B)Y, (a B)X, (a B)Y) can be calculated as follows.In a Cartesian co-ordinate system,X B=X A+L AB * cos(θ) and Y B=Y A+L AB * sin(θ) Differentiating the above position analysis formulae with respect to time, the formulae for velocity analysis can be derived.(V B)X=(V A)X - L AB * sin(θ)*ωand (V B)Y=(V A)Y +L AB*cos(θ)*ωDifferentiating again, the formulae for analyzing the acceleration of the point B can be derived.(a B)X= (a A)X - L AB* sin(θ)*ε - L AB* cos(θ)*ω2and(a B)Y = (a A)Y + L AB* cos(θ)*ε -L AB* sin(θ)*ω2These six formulae can be programmed in a subroutine. In the TRUE BASIC computer language, any subroutine must begin with statement SUB subroutine-name(table of parameters) and end with statement END SUB. Let us name the subroutine LINK. Then the LINK subroutine is as follows:SUB LINK(XA,YA,V AX,V AY,AAX,AAY,Q,W,E,LAB,XB,YB,VBX,VBY,ABX,ABY) LET XB=XA+LAB*COS(Q)LET YB=YA+LAB*SIN(Q)LET VBX=V AX-LAB*SIN(Q)*WLET VBY=V AY+LAB*COS(Q)*WLET ABX=AAX-LAB*SIN(Q)*E-LAB*COS(Q)*W^2LET ABY=AAY+LAB*COS(Q)*E-LAB*SIN(Q)*W^2END SUBEvery evaluating statement must begin with LET. In order to facilitate the understanding of the program, parameters should have easily-recognized names. For example, (V A )X is named V AX. The table of the parameters corresponds to (X A , Y A , (V A )X , (V A )Y , (a A )X , (a A )Y , θ, ω, ε, L AB , X B , Y B , (V B )X , (V B )Y , (a B )X , (a B )Y ). After the subroutine is called, the kinematic parameters of the point B will be known. 3.3.2 The RRR SubroutineIn the RRR group shown inthe kinematic parameters of the points A and C and the lengths of links AB and CB are known. The positions, angular velocities and calculated as follows. When X A , Y A , X C , Y C , L AB determined, there are two assembly modesfor this group, as shown in Fig.3-8, one in solid lines and the other in dashed lines. On the link CB, X B =X C +L CB *cos(θCB ) and Y B =Y C +L CB *sin(θCB )On the link AB, X B =X A +L AB *cos(θAB ) and Y B =Y A +L AB *sin(θAB )Combining these two sets of equations, one obtains:X L X L Y L Y L C CB CB A AB AB CCB CB A AB AB +=++=+⎧⎨⎩*cos()*cos()*sin()*sin()θθθθ ----------------------------(3-2) There are two unknowns, θAB and θCB , in this set of equations. Since there are two assembly modes for this group, there will be two sets of solutions. Although some mathematical skill can be used to solve the above trigonometric non-linear equations to obtain two sets of formulae for θAB and θCB , the calculation process is tedious and the formulae derived would be very complicated. It is hard to judge which set of formulae corresponds to a specific assembly mode. The following is a simple method to overcome this difficulty.(a) L AC =()()X X Y Y C A C A -+-22(b) cos θAC =(X C -X A )/L AC and sin θAC =(Y C -Y A )/L ACThe subroutine may be used for any combinations of the positions of points A Fig.3-8and C. Note that sin θAC may not be equal to ()12-cos θAC since sin θAC may be negative. The magnitude of θAC can be calculated according to the values of both cos θAC and sin θAC by the ANGLE function in TRUE BASIC. Note again that θ may not be equal to ATN(sin θ/cos θ) since θ may be greater than π/2 and less than 3*π/2, whereas the value obtained from the ATN function is only from -π/2 to +π/2. Note: θAC is 180︒ different from θCA .(c) cos θBAC =()()L L L L L AB AC CB AB AC 2222+-/** Since 180︒>θBAC >0︒, sin θBAC =()12-cos θBAC . If L AC >(L AB +L CB ), then cos θBAC >1. This means that the distance L AC between the two outer points is greater than the sum of L AB and L CB . If L AC <|L AB -L CB |, then cos θBAC <(-1) and the distance L AC is less than the difference between L AB and L CB . In these cases, the RRR dyad can not be assembled. The calculation of ()12-cos θBAC will fail and computation will be stopped.(d) As mentioned before, there are two assembly modes for the RRR group. For the assembly mode shown by solid lines, θAB =θAC -θBAC . During motion of the mechanism, the assembly mode does not change as a result of change in position.(e) X B =X A +L AB *cos(θAB ) and Y B =Y A +L AB *sin(θAB )(f) cos θCB =(X B -X C )/L CB and sin θCB =(Y B -Y C )/L CBThe magnitude of θCB can be calculated according to the values of both cos θCB and sin θCB by the ANGLE function in TRUE BASIC.Velocity analysis can be progressed only after the position analysis is finished. The angular velocities, ωAB and ωCB , of the links AB and CB can be found by differentiating Eqs.(3-2) with respect to time. ()*sin()*()*sin()*()*cos()*()*cos()*V L V L V L V L C X CB CB CB A X AB AB AB C YCB CB CB A Y AB AB AB -=-+=+⎧⎨⎩θωθωθωθω -------------(3-3) Both velocity and acceleration equations of a grade II Assur group are dualistic linear equations. The explicit expressions for ωAB and ωCB can be found easily by solving the two equations simultaneously.By differentiating Eqs.(3-3) with respect to time, another set of dualistic linear equations with two unknowns, i.e. the angular accelerations εAB and εCB ofthe links AB and CB, is derived. The explicit expressions for εAB and εCB can be found easily by solving the two equations simultaneously.Using the above explicit expressions, not the equations, the subroutine named RRR for kinematic analysis of the RRR group can be written as follows:SUB RRR(XA, YA, V AX, V AY, AAX, AAY, XC, YC, VCX, VCY, ACX, ACY, LAB, LCB, QAB, WAB, EAB, QCB, WCB, ECB)LET LAC=SQR((XC-XA)^2+(YC-YA)^2)LET COSQAC=(XC-XA)/LACLET SINQAC=(YC-YA)/LACLET QAC=ANGLE(COSQAC,SINQAC)LET COSQBAC=(LAB^2+LAC^2-LCB^2)/(2*LAB*LAC)LET SINQBAC=SQR(1-COSQBAC^2)LET QBAC=ANGLE(COSQBAC,SINQBAC)LET QAB=QAC-QBACLET XB=XA+LAB*COS(QAB)LET YB=YA+LAB*SIN(QAB)LET COSQCB=(XB-XC)/LCBLET SINQCB=(YB-YC)/LCBLET QCB=ANGLE(COSQCB,SINQCB).......................................LET WAB=....................LET WCB=.............................................................LET EAB=.......................LET ECB=.......................END SUBAttention should be paid to the sequence of the revolute's letters in the table of the parameters when the subroutine is called. For this subroutine, the three letters A, B and C are arranged in CCW.3.3.3 The RPR SubroutineShown in Fig.3-9(a) is an RPR Assur group. The revolutes A and C are outer revolute pairs. The eccentric AB is perpendicular to guide-bar BD. The kinematic parameters of the centers, A and C, of the two outer revolute pairs and the length of eccentric AB are known. There are two assembly modes for this group. One is shown in solid lines, the other in dashed lines. The angular position, angular velocity and angular acceleration of the guide-bar BD (θBD, ω, ε) can be calculated as follows:L AC =()()X X Y Y C A C A -+-22, cos θAC =(X C -X A )/L AC ,sin θAC =(Y C -Y A )/L AC ,L BC =L L AC AB 22-.If L AC <L AB , then the group can not be assembled. In this case, the calculation ofL L AC AB 22- will fail and the computation will be stopped.θACB =tg L L AB BC -⎛⎝ ⎫⎭⎪1, θBD = θAC +M* θACB , and θAB = θBD -M*π/2where M is the coefficient of the assembly mode. For the solid mode, M=+1. For the dashed mode, M=-1. During motion of the mechanism, the assembly mode does not change as a result of change in position. We can determine the value of M according to the assembly mode at any angle of the driver.From Fig.3-9, we haveX X L X L L Y Y L Y L L C B BC BD A AB AB BC BD CB BC BD A AB AB BC BD =+=++=+=++⎧⎨⎩cos()cos()cos()sin()sin()sin()θθθθθθ Differentiating the above equations with respect to time results in--+=--+=-⎧⎨⎩()cos()*()()()sin()*()()Y Y VLBC V V X X VLBC V V C A BD C X A XCA BD C Y A Y ωθωθ -------------------(3-4) where VLBC is the derivative of L BC with respect to time.Solving the dualistic linear equations Eqs.(3-4) simultaneously, the explicit(a) (b) Fig.3-9expressions for the two unknowns ω and VLBC can be found.Differentiating Eqs.(3-4) with respect to time (note that VLBC is a variable), another set of dualistic linear equations with two unknowns (one of the unknowns is ε) is derived. The explicit expression for ε can be found easily by solving the two equations simultaneously.The subroutine for the kinematic analysis of the RPR group, which we will name RPR, can be written as follows:SUB RPR(M, XA, YA, V AX, V AY, AAX, AAY, XC, YC, VCX, VCY, ACX, ACY, LAB, QBD, W, E)LET LAC=SQR((XC-XA)^2+(YC-YA)^2)..........................................LET QBD=QAC+M*QACB..........................................LET W=.....................................................................LET E= ..........................END SUBIf L AB=0, then the RPR group in Fig.3-9(a) is simplified into another RPR group shown in Fig.3-9(b). For the RPR group in Fig.3-9(b), L AB=0 and θACB=0. The value of M can be set as any value.Kinematic analysis subroutines for other grade II Assur groups, e.g. RRP, PRP groups in Tab.2-2, can also be derived and established in a similar method. 3.3.4 Main ProgramTo analyze any mechanism, a main program is required. In the main program, suitable kinematic analysis subroutinesAssur groups.Example 3-4The six-bar linkage shown ina constant angular velocity ω1ofknown dimensions of the mechanism are:XX B=41mm, Y B=0, X F=0, Y F=-34m, L ED=14mm, LL BA=28mm, ∠ADC=35︒, L DC=15mm, L FGFig.3-10 program is required to analyze the output motions oflink FG and point G. The mechanism will be analyzed for the whole cycle when thedriver ED rotates from 0︒ to 360︒ with a step size of 5︒.Solution:(a) Group dividingThe composition of this mechanism has been analyzed in Sec.2.5.3. The types and the assembly orders of Assur groups are listed in Table 2-3 of Chapter 2. In this linkage, link ED is the driver. Links 3 and 2 forms an RRR dyad. After this dyad is connected to the driver and the frame, the motion of both links 3 and 2 are determined. Thus we can determine the motion of point C on the link 3. Rocker 4 and block 5 forms a RPR dyad. This dyad can be assembled only after the motion of the point C is determined.(b) Main programThe main program for kinematic analysis of this linkage mechanism can be written as follows:REM The main program for the linkage mechanism in Fig.3-10FOR Q1=0 TO 360 STEP 5CALL LINK(0, 0, 0, 0, 0, 0, Q1*PI/180, 10, 0, 14, XD, YD, VDX, VDY,ADX, ADY)CALL RRR(XD, YD, VDX, VDY, ADX, ADY, 41, 0, 0, 0, 0, 0, 39, 28, Q3, W3, E3, Q2, W2, E2)LET QDC=Q3+35*PI/180CALL LINK(XD YD, VDX, VDY, ADX, ADY, QDC, W3, E3, 15, XC, YC,VCX, VCY, ACX, ACY)CALL RPR(0, 0, -34, 0, 0, 0, 0, XC, YC, VCX, VCY, ACX, ACY, 0, Q4, W4,E4)CALL LINK(0, -34, 0, 0, 0, 0, Q4, W4, E4, 55, XG, YG, VGX, VGY, AGX,AGY)PRINT Q1, Q4*180/PI, W4, E4, XG, YG, VGX, VGY, AGX, AGYNEXT Q1ENDThe subroutine for the first Assur group RRR should be called before that for the second Assur group RPR is called. In the first Assur group RRR, the point D should be a determined point. So we use the first CALL LINK statement to calculate the kinematic parameters of the point D before RRR is called. The parameter PI in the first CALL line is set to πby TRUE BASIC automatically. Emphasis should be put on the assembly mode, the sequence and the correspondence of parameters in the table of parameters when calling a subroutine. Corresponding data are transferred according to the sequence, not according to thename.As mentioned before, an RRR group has two assembly modes, as shown in Fig.3-8. The RRR subroutine is written for the assembly mode shown in solid lines. In order to use the RRR subroutine, the revolutes D, A and B of the RRR dyad in Fig.3-10 must correspond to the revolutes A, B and C of the RRR dyad in Fig.3-8, respectively.In the second Assur group RPR, the revolute centre C should be a determined point. So the second CALL LINK statement must be used to calculate the kinematic parameters of the point C after RRR is called and before RPR is called.The main program ends with the statement END after which all subroutines are listed in any order. Putting the related subroutines(LINK, RRR, RPR) after the END statement of the main program and running it on a computer, produces the3.3.5 Check on the Output DataFaced with a vast amount of digits printed on the screen, it is hard to judge whether or not the output results are correct. The output values from the analytical methods can be checked as follows. For some non-special position of the mechanism, try to draw the kinematic diagram of the linkage mechanism as exactly as possible. Measure the X and Y coordinates of the output points and/or the angular position φ of the output link. Then the measured data are compared with the output position data obtained by the analytical method. Minor differences between the measured values and the analyzed values are acceptable and can be considered as drawing errors and measuring errors. If the output position at the non-special position of the mechanism is correct and the output position data change smoothly during the whole cycle, then the position analysis can be considered to be correct.After the output position data are checked, the output velocity data obtained in the analysis methods can be checked by the instant centers method or by other methods.A simpler way to check output velocity is to examine the qualitative nature of the data. For example, if the displacement is increasing, the corresponding velocity must be positive; otherwise, negative. When the displacement reaches its limit, the corresponding velocity must be zero.Similarly, if the velocity is increasing, the corresponding acceleration must be positive; otherwise, negative. When the velocity reaches its limit, the corresponding。

中英文单词对照

Chapter 1 Introduction 绪论双语教材bilingual textbook机械原理theory of machine and mechanisms机械学mechanology机构学mechanism机械设计及理论machine design and theory of machine机械machinery机器machine机构mechanism内燃机internal-combustion engine活塞piston曲轴crankshaft构件link, member零件part, element螺母nut螺栓bolt垫片washer轴承bearing轴瓦bearing, bush连杆coupler, connecting rod机械设计的步骤mechanical design procedure微型机械（微米级）micromachine小型机械minimachine机器组成constitution of machine机械运动学kinematics of machinery机械动力学dynamics of machinery过程process机械设计machine design国际机械原理联合会international federation for theory of machines and mechanisms单缸四冲程内燃机one-cylinder four-stroke cycle combustion engine吸气行程intake stroke压缩行程compression stroke压力行程power stroke排气行程exhaust strokeChapter 2 Structural Analysis of Planar Mechanisms 平面机构的结构分析机构组成constitution of mechanism机构分析structure analysis原动机prime machine, prime power原动件driving link, input link从动件driven link, output link机架frame, fixed link连架杆link connected with frame, side link相对运动relative motion接触contact运动副kinematical pair运动副的分类classification of kinematical pairs低副lower pair高副higher pair转动副turning pair, revolute pair移动副sliding pair, prismatic pair滚滑高副sliding-turning pair二副杆binary link三副杆ternary link平面机构plane mechanism空间机构spatial mechanism运动链kinematical chain平面运动链plane chain空间运动链spatial chain闭链closed chain开链unclosed chain, open chain自由度degree of freedom, mobility约束constraint虚约束overconstraint redundant constraint局部自由度partial degree of freedom, passive degree of freedom,redundant degree of freedom复合铰链multiple pin joints, compound hinges机构运动简图schematic diagram of mechanism, kinematic sketch颚式破碎机jaw crusher machine长度比例尺length scale刚性构件rigid link柔性构件flexible link活动构件moving link高副低代replacement of higher pair by lower pair机构的结构分析structure analysis of mechanism杆组link groupII级杆组class II link groupIII级杆组class III link group复杂机构complex mechanism等效机构equivalent mechanism平面机构自由度计算公式Grueble criterion for degree of freedom of plane mechanism空间机构自由度计算公式Kutzback criterion for degree of freedom of spatial mechanismChapter 3 Kinematic Analysis of Planar Mechanisms 平面机构的运动分析图解法graphical method相对运动图解法graphical method of relative motion瞬心法velocity analysis by instantaneous centers解析法analytical method运动分析kinematic method线位移displacement, linear displacement线速度velocity, linear velocity线加速度acceleration, linear acceleration转角angle角位移angular displacement角速度angular velocity角加速度angular acceleration位移方程displacement equation速度方程relative velocity equation加速度方程relative acceleration equation绝对速度absolute velocity相对速度relative velocity牵连速度implicative velocity, velocity of the base point法向速度normal velocity切向速度tangential velocity哥式加速度coriolis component of acceleration, coriolis acceleration 位移分析displacement analysis速度分析velocity analysis速度影像velocity image加速度影像acceleration image加速度分析acceleration analysis重合点coincident points瞬心instant center, instantaneous center速度瞬心velocity center绝对瞬心absolute center相对瞬心relative center瞬心多边形instantaneous center polygon用运动副直接连接的两构件瞬心prime center三心定理aronhold-kennedy theorem极点pole速度比例尺velocity scale加速度比例尺acceleration scale速度多边形velocity polygon加速度多边形acceleration polygon矢量vector标量scalar大小与方向magnitude and direction直角坐标系rectangular coordinate封闭矢量环closed vector loop矩阵matrix线性代数方程linear algebraic equation线性方程组linear equations非线性方程组non-linear equations数学模型mathematical model计算机辅助设计computer aided design (CAD)计算机程序computer programChapter 4 Force Analysis of Planar Mechanisms 平面机构的力分析力分析force analysis静力分析static analysis动力分析dynamic analysis作用力applied force力矩moment驱动力driving force阻抗力resistance force重力gravity惯性力inertia force约束反力reactive force, reaction分离体free-body diagram二力构件two-force member三力构件three-force member力作用线action line of force平衡方程equation of equilibrium摩擦friction摩擦系数coefficient of friction当量摩擦系数equivalent coefficient of friction干摩擦dry friction平面摩擦friction on plane斜面摩擦friction on inclined plane矩形螺纹摩擦friction on square-threaded screw三角螺纹摩擦friction o v-threaded screw滑动摩擦sliding friction接触表面contacting surface磨损wear摩擦力friction force摩擦角friction angle当量摩擦角equivalent friction angle总反力total resultant force轴颈摩擦pin friction, friction in journal bearing摩擦圆friction circle达朗伯原理d’alember’s principle惯性力矩inertia torque, moment of inertia质心center of mass, mass center转动惯量mass moment of inertia离心力centrifugal force水平力horizontal force切向力tangential force法向力normal force自锁self-locking机械效率mechanical efficiency自锁机构self-locking mechanism输入功率input power输出功率output powerChapter 5 Synthesis of Planar Linkages 平面连杆机构及其设计运动综合精确综合近似综合型综合数综合尺度综合精度结构误差按行程速比系数综合四杆机构按连杆的对应位置综合四杆机构按连架杆的对应位置综合四杆结构按连杆曲线综合四杆机构刚体导引函数发生轨迹生成连杆机构linkage平面连杆机构plane linkage空间连杆机构spatial linkage四杆机构four-bar linkage曲柄摇杆机构crank-rocker linkage双曲柄机构double-crank linkage双摇杆机构double-rocker linkage曲柄滑块机构slider-crank linkage曲柄摇杆机构rocking-block linkage摆动导杆机构rocking guide-bar linkage转动导杆机构移动导杆机构正弦机构正切机构双滑块机构双转块机构平行四边形机构曲柄存在的条件压力角pressure angle传动角transmission angle极位夹角angle between the limiting position极限位置extreme position, limiting position行程速比系数coefficient of travel speed variation, advance to return time ratio 急回特性quick-return characteristics急回运动quick-return motion急回机构quick-return mechanism死点dead point机构演化机构变异机架变换移动定轴转动一般平面运动Chapter 6 Design of Cam Mechanisms 凸轮机构及其设计凸轮cam高速凸轮high-speed cam从动件follower滚子roller滚子半径radius of roller滚子中心center of roller平底长度length of flat-face直动从动件translating follower摆动从动件oscillating follower滚子从动件roller follower尖顶从动件knife-edge follower平底从动件flat-faced follower, flat follower曲底从动件spherical-faced follower对心直动从动件盘形凸轮机构disk cam with radial translating roller follower对心直动尖顶从动件盘形凸轮机构disk cam with radial translating knife-edge follower偏置直动从动件盘形凸轮机构disk cam with offset translating roller follower偏置直动滚子从动件盘形凸轮机构摆动滚子从动件盘形凸轮机构disk cam with offset oscillating follower摆动尖顶从动件盘形凸轮机构摆动平底从动件盘形凸轮机构摆动滚子从动件盘形凸轮机构直动滚子从动件盘形凸轮机构摆动滚子从动件圆柱凸轮机构运动规律follower motion多项式运动规律polynomial motion一阶多项式运动规律二阶多项式运动规律3-4-5次多项式运动规律等速运动规律uniform motion, straight-line motion等加速、等减速运动规律parabolic motion, constant acceleration motion 三角函数运动规律trigonometric function motion摆线运动规律cycloidal motion简谐运动规律simple harmonic motion正弦运动规律sine acceleration motion, sine motion余弦运动规律cosine acceleration motion, cosine motion组合运动规律combination of follower motion位移线图displacement diagram位移方程displacement equation速度线图velocity diagram加速度线图acceleration diagram盘形凸轮disk cam, plate cam移动凸轮translating cam, wedge cam等径凸轮等宽凸轮共轭凸轮圆柱凸轮圆锥凸轮反转法原理principle of inversion包络线envelope实际廓线基圆base circle偏距offset偏距圆offset circle理论廓线基圆prime circle理论廓线pitch curve实际廓线cam profile曲率curvature凸轮廓线的曲率半径运动失真尖点sharp point, cusp外凸曲线内凹曲线滚子中心点开始点initial point升程rise travel回程return travel行程stroke从动件位移follower displacement凸轮转角cam angle停顿dwell升程运动角rise angle, angle of ascent回程运动角return angle, angle of decent休止角repose angle, angle of dwell刀具中心轨迹刚性冲击rigid impulse柔性冲击flexible impulseChapter 7 Design of Gear Mechanisms 齿轮机构及其设计齿轮机构gear mechanism平行轴齿轮parallel gears相交轴齿轮intersecting gears交错轴齿轮skew gear圆柱齿轮cylindrical gear锥齿轮bevel gear蜗轮warm gear蜗杆warm直齿圆柱齿轮spur gear斜齿圆柱齿轮helical gear齿廓曲线tooth profile齿廓啮合基本定律fundamental law of toothed gearing, law of gearing 发生线generating line渐开线involute渐开线性质involute property渐开线函数involute function渐开线方程involute equation中心距distance of centers标准中心距standard distance of centers实际安装中心距mounted distance of centers节点pitch point节圆pitch circle分度圆reference circle, standard pitch circle基圆base circle齿顶圆addendum circle齿根圆dedendum circle齿数number of teeth模数module齿距circular pitch基节base pitch法节normal pitch齿厚tooth thickness齿槽宽width of space, space width任意圆周上的齿厚齿顶高addendum齿根高dedendum全齿高whole depth顶隙clearance侧隙backlash齿顶高系数coefficient of addendum顶隙系数coefficient of clearance基圆直径diameter of clearance分度圆直径diameter of reference circle节圆直径diameter of pitch circle齿顶圆直径diameter of addendum circle齿根圆直径diameter of dedendum circle公法线长度length of common normal小齿轮pinion外齿轮external gear齿条rack内齿轮internal gear, ring gear啮合mesh, engaging啮合点engaging point, meshing point啮合线line of action啮合角meshing angle, angle of obliquity正确啮合条件condition of correctly engaging重合度contact ratio滑动系数slip ratio齿轮加工forming of gear teeth, gear manufacture成形法form milling范成法generating盘形刀具disk milling cutter指形刀具finger cutter齿条刀具rack-shaped cutter干涉inerference根切undercutting不发生根切的最少齿数minimum number of teeth to avoid undercutting标准齿轮standard gear变位齿轮nonstandard gear, modified gear变位系数coefficient of offset变位offset正变位positive offset负变位negative offset正变位齿轮positive offset modified gear负变位齿轮negative offset modified gear不发生根切的最小变位系数smallest coefficient of offset to avoid undercutting 分度圆分离系数齿顶高降低系数无侧隙啮合方程分度圆分离方程基圆柱渐开面渐开螺旋面螺旋thread螺旋角helix angle左旋left-hand右旋right-hand法面normal plane端面transverse plane, plane of rotation法向参数normal parameter端面参数transverse parameter法向压力角normal angle端面压力角transverse angle法向模数normal module端面模数transverse angle法向基节normal base pitch端面基节transverse base pitch法向齿厚normal tooth thickness端面齿厚transverse tooth thickness法向齿顶高系数coefficient of normal addendum端面齿顶高系数coefficient of transverse addendum法向顶隙系数coefficient of normal radical clearance端面顶隙系数coefficient of transverse radical clearance 法向重合度normal contact ratio端面重合度transverse contact ratio当量齿轮equivalent spur gear当量齿数number of teeth of the equivalent spur gear 法向力normal force径向力radial force轴向力axial force人字齿轮herringbone gear螺旋齿轮crossed helical gear阿基米德蜗杆achimedes worm蜗杆直径系数quotient of warm diameter主截面main section螺纹升角lead angle直齿锥齿轮straight-tooth bevel gear曲齿锥齿轮spiral bevel gear球面渐开线spherical involute轴角shaft angle齿顶圆锥face cone节圆锥pitch cone齿根圆锥root cone节锥角pitch cone angle锥距cone distance锥顶common apex顶锥角face angle根锥角root angle齿顶角addendum angel齿根角dedendum angle背锥back cone平面锥齿轮crown gear准双曲面锥齿轮hypoid gearChapter 8 Gear Trains 轮系及其设计轮系gear train定轴轮系ordinary gear train单式轮系simple gear train复式轮系compound gear train回归轮系reverted gear train周转轮系epicyclic gear train差动轮系differential gear train行星轮系planetary gear train混合轮系compound epicyclic gear train, combined gear train串联轮系tandem combined gear train封闭轮系closed combined gear train传动比angular velocity ratio, speed ratio太阳轮sun gear行星轮planet gear系杆planet carrier, arm转化机构inversion gear train, converted gear train轮系效率efficiency of gear train少齿差行星传动planetary gear train with small tooth difference摆线针轮cycloidal-pin wheel摆线齿轮cucloidal gear谐波传动harmonic drive波数number of wave波发生器wave generator刚轮rigid circular spline柔轮flex spline, flexible wheel惰轮idle gear平行齿轮传动parallel move gearing减速器reducerChapter 9 Introduction of Screws, Hook’s Couplings and Inermittent Mechanisms 螺旋机构、万向联轴器、间歇运动机构简介螺旋传动 screw drive螺旋机构 screw mechanism往复移动 rectilinear motion差动螺旋机构 differential screw mechanism滚珠螺旋机构 roller screw mechanism万向联轴器 universal joints, hook ’s coupling单万向联轴器 single universal joint十字叉 cross piece叉头 yoke双万向联轴器 double universal joints间歇运动机构 intermittent motion mechanism自动机械 automatic machinery间歇转动 intermittent rotation棘轮机构 ratchet mechanism棘轮 ratchet wheel, ratchet棘爪 pawl无声棘轮 silent ratchet wheel槽轮机构 Geneva wheel mechanism锁紧盘 locking plate内槽轮机构 inverse Geneva wheel mechanism运动系数 action coefficient蜂窝煤机 beehive coal tool machine不完全齿轮机构 intermittent gear mechanism分度凸轮机构 indexing cam mechanism圆柱形分度凸轮机构 cylindrical cam indexing mechanism蜗杆形分度凸轮机构 worm-cam indexing mechanism(1) Gear Teeth FormingThere are many methods of forming the teeth of gears, such as shell molding, investment casting, permanent-mold casting, die casting, centrifugal casting. Gears can also be formed by using the power-metallurgy process or by using extrusion; a single metal bar can be formed and then sliced into gear. Usually gears are cut with either form cutters or generating cutters.(2) Advantages and Disadvantages of Helical GearsHelical gears are more expensive than spur gears but offer some advantages. They operate quieter than spur gears because of smooth and gradual contact between their angle surfaces as the teeth come into mesh. Spur gear teeth mesh along their entire face width at once; the sudden impact of tooth on tooth causes vibration. Also, for the same gear diameter and module, a helical gear is stronger due to the slightly thicker tooth form in a plane perpendicular to the gear axis.The one of the disadvantages of helical gear is that they produce an axial thrust force which is harmful to the bearings. Therefore, the helix angle is limited from οο15~8.When motion is to be transmitted between shafts which are not parallel, the spur gears can not be used. Two crossed-helical gears of the same hand can be meshed with their axed at an angle, the helix angles can be designed to accommodate any skew angle between the nonintersecting shafts.(3)Characteristics of Worm GearsWorm and worm gear are used to transmit motion between two shafts which are nonparallel, nonintersecting, usually at a shaft angles of90. The worm gears have many advantages, such as larger gear ratios, small package, carrying high loads. Perhaps the major advantage of the worm set is that it can be designed to be impossible to backdrive, that is to say, it can be designed as a self-locking worm set. Therefore, worm gears are most widely used in industry. The major disadvantages is that the efficiency is lower, usually at 40% to 85%. The friction loss may result in overhearing and serious wear.(4)Classification of Gear TrainsA gear train is a combination of gears used to transmit motion from one shaft to another. Even a single pair of gear is, strictly speaking, a gear train, though the term usually suggests that there are three or more moving gears. The gear train becomes necessary when it is required to obtain large speed ratio with a small space.Gear-box is used in automobile. There are many pairs of gears to transmit different motion. Quartz watch has gear trains in which the hour hand, minute hand and second hand rotate as their definite gear ratios to indicate the times.A gear train is any collection of two or more meshing gears, such as spur gears, bevel gears, worm gears and their combination of different kinds of gears. If all gear axes remain fixed relative to the frame, train is an ordinary gear train. If one of the gear axes rotates to the frame, this gear train is an epicycle gear train.The designer is frequently confronted with the problem of transferring power from one shaft to another while maintaining a definite ratio between the velocities of rotation of the shafts. Another requirement might be the transmission of a specified angular for this purpose, which will operate quietly and with very low friction losses. Smooth and vibrationless action is secured by giving the proper geometric shape to the outline of the teeth. The proportions of the gear tooth, as well as the sizes of the teeth, have been standardized. This procedure has simplified design calculations and has reduced the required number of cutting tools to a minimum. The proper material must be selected to obtain satisfactory strength, fatigue, and wear properties. Ease of manufacture and ease of inspection are necessary if production costs are to be kept at their lowest level. All these problems must be taken into account by the designer.滚动轴承Rolling bearing一、轴承滚动体rolling element保持架cage, retainer内圈inner ring外圈outer ring滚动轴承rolling bearing单列轴承single row bearing双列轴承double row bearing球轴承ball bearing深沟球轴承deep groove ball bearing推力轴承thrust bearing推力球轴承thrust ball bearing单列双向推力球轴承single row double-direction thrust ball bearing双列单向推力球轴承double row single-direction thrust ball bearing角接触轴承angular contact bearing调心轴承self-aligning bearing滚子轴承roller bearing圆柱滚子轴承cylindrical roller bearing圆锥滚子轴承tapered roller bearing滚针轴承needle roller bearing推力滚子轴承thrust roller bearing向心球轴承radial ball bearing角接触轴承angular contact bearing调心轴承self-aligning bearing向心轴承radial bearing角接触向心轴承angular contact radial bearing单列深沟球轴承是球轴承中最普遍的种类，应用及其广泛。

chapter3_new

Mechanisms and Machine Theory
2
CHAPTER 3 KINEMATIC ANALYSIS OF MECHANISMS (c ) Acceleration analysis: to calculate the dynamic forces. Methods (1) Graphical method (a) Geometrical method for position; (b) Instant centre (瞬心) method for velocity; (c) Vector equation (矢量方程) method for velocity and acceleration.
A pair of coincident (重合) points of two links. The absolute velocities (绝对速度) of which are the same, in both magnitude (大小) and direction and the Relative velocity is zero.
Mechanisms and Machine Theory
15
CHAPTER 3 KINEMATIC ANALYSIS OF MECHANISMS
3.2.4 Theorem of Three Centres (三心定理，also known as Aronhold-Kennedy Theorem)
Mechanisms and Machine Theory
7
CHAPTER 3 KINEMATIC ANALYSIS OF MECHANISMS
3.2 Velocity Analysis by the Method of Instant Centres (瞬心法)

Exploring SolidWorks Motion for Kinematic Analysis

Exploring SolidWorks Motion forKinematic AnalysisIntroduction:SolidWorks Motion is a powerful software tool that enables engineers and designers to simulate and analyze the kinematics of their mechanical systems. Whether it's evaluating the motion of a simple mechanism or conducting a comprehensive analysis of a complex assembly, SolidWorks Motion provides the necessary tools to understand how components move and interact with each other.Kinematics, in simple terms, is the study of motion without considering the forces that cause it. It helps engineers understand how different parts of a mechanism move relative to each other, thereby aiding in the optimization of design and functionality.SolidWorks Motion Features:1. Kinematic Analysis:SolidWorks Motion offers a range of analysis tools to evaluate the kinematic behavior of assemblies. It allows users to define and simulate the motion of individual components or the entire assembly. By applying various motion types such as translational, rotational, and combined, engineers can assess the movement, velocity, acceleration, and jerk of different parts.For example, if you have a mechanism with rotating components such as gears or cams, SolidWorks Motion can calculate the angular positions and velocities accurately. It helps identify constraints or interference issues, enabling designers to make necessary modifications for optimal performance.2. Motion Studies:Motion Studies in SolidWorks Motion provide a comprehensive way to analyze the kinematics of assemblies. Users can create and simulate multiple scenarios to understandhow different design variations affect the motion of a mechanism. By defining relationships, forces, and motion inputs, engineers can observe and compare the behavior of components under various conditions.Motion studies allow for the creation of time-based animations, enabling visualizations of how parts move and interact. This feature is particularly beneficial in communicating design concepts, identifying potential collisions, and validating the functionality of a mechanical system.3. Contact Detection and Collision Analysis:SolidWorks Motion incorporates robust algorithms for detecting and analyzing interferences during the motion simulation of an assembly. This capability helps engineers identify potential collisions or overconstraints that might lead to unintended behavior or failure.By incorporating contact detection and collision analysis, the software allows usersto adjust their designs, modify constraints, or add protective features to prevent collisions. It ensures that your mechanism operates smoothly and safely.4. Motion Optimization:SolidWorks Motion also offers optimization tools to enhance the kinematic performance of designs. This feature enables engineers to set up design parameters and constraints, allowing the software to automatically adjust the design to achieve desired outcomes.By utilizing optimization techniques, users can fine-tune parameters, such as dimensions or constraints, to optimize the efficiency, speed, or accuracy of a mechanism's motion. This leads to cost savings, improved performance, and less iteration during the design process.5. Real-time Analysis:One of the key advantages of SolidWorks Motion is its ability to provide real-time analysis of kinematic behavior. Engineers can observe the motion simulation in real-time, allowing for quick assessments and iterative modifications.The real-time analysis feature enhances the collaboration between design teams as they can share the simulation results during the design review process. It helps in identifying any design or performance issues early on, reducing time and rework.Conclusion:SolidWorks Motion offers a comprehensive set of tools for kinematic analysis, enabling engineers to simulate, analyze, and optimize the motion of mechanical systems. By utilizing these features, designers can gain valuable insights into the behavior of their assemblies, optimize performance, and make informed design decisions.From kinematic analysis to motion studies, contact detection to optimization, and real-time analysis to improve collaboration, SolidWorks Motion provides a powerful platform to explore the kinematics of mechanical systems. By utilizing this software, engineers can enhance the quality, efficiency, and reliability of their designs, leading to improved product performance and customer satisfaction.。

腱绳驱动仿人灵巧手运动分析

NEW PRODUCT NEW TECHNOLOGY0 引言伴随着工业及科技领域的蓬勃发展，中国对航空航天、核工业等战略性产业愈加重视。

在这些工业场景中，工作人员通常需要在极端、危险的条件下进行作业，对人身体健康会产生较大危害。

为此，可替代技术人员进入此类极端工业场景完成复杂工作任务的智能机器人应运而生，其末端执行器的选择直接影响了机器人的工作效率。

作为结合了仿生学新型末端执行器[1，2]的灵巧手，拥有灵巧性高、适应性强、可完成多种不同类型的复杂操作等优点，成为近年来机器人领域研究的热点。

仿人灵巧手可分为全驱动仿人灵巧手和欠驱动仿人灵巧手[3-7]2类。

针对欠驱动灵巧手的发展和研究，美国宇航局（NASA）对在航天领域应用的空间机器人研制了早期最为经典的一种欠驱动灵巧手Robonaut Hand[8]，此后又与通用汽车合作对上一代灵巧手进行优化研制了第二代Robonaut Hand。

Catalano M G等[9]研制了PISA/IIT SoftHand，这种手仅用单一舵机驱动整个具有19个自由度的灵巧手，其很好地利用了腱绳驱动方式的优势，为腱绳驱动灵巧手提供了思路。

徐昱琳等[10]研制的SHU-Ⅱ采用轻质腱绳驱动并将6个直流电动机内置于手掌，其中5个电动机分别控制各手指弯曲运动，余下的1个电动机控制拇指侧摆，具有较大的运动抓取空间。

基于此，本文设计了一种腱绳驱动仿人灵巧手，先对其进行运动学分析，再求出其雅克比矩阵，分析手指动力学性能和运动空间，为仿人灵巧手提供控制依据和理论。

1 仿人灵巧手的总体设计本文灵巧手依据人手作为仿生对象，根据人体手部腱绳驱动仿人灵巧手运动分析王峥宇1 张立1 陈耀轩1 梅杰1，2 陈定方1，21武汉理工大学交通与物流工程学院武汉 430063 2武汉理工大学智能制造与控制研究所武汉 430063摘要：针对在极端环境下代替人工进行作业的需求，文中设计了一种由腱绳驱动的灵巧手作为高效机器人末端执行器。

kinematic Analysis

3Kinematic AnalysisThe kinematic constraint equations corresponding to the natural coordinates were explained in detail in Chapter 2, both for planar and three-dimensional multibody systems. They were then compared to other types of coordinates. Attention was also given to the main sources of constraint equations with natural coordinates: rigid body constraints, joint constraints, and the optional definition of relative or joint coordinates.In this chapter we will make use of those constraint equations to solve what is usually called kinematic problems, namely, the initial position or assembly problem, the finite displacement problem, and the velocity and acceleration analysis. The first two problems require an iterative solution of a system of nonlinear equations. Some special techniques to improve the convergence will be explained. Special attention will be addressed to the important case of over constrained multibody systems or, in general, to systems with non-independent constraint equations. The last section of this chapter is devoted to the case of non-holonomic joints.3.1 Initial Position ProblemThe initial position problem was defined in Section 1.3. It basically consists of determining the position of all the bodies in the system by knowing the positions of the fixed and the input bodies which can also be called guided or driven ele-ments. Mathematically, the initial position problem is reduced to determining from the known coordinates corresponding to the input elements the vector of dependent coordinates that satisfies the nonlinear system of constraint equa-tions. Note that the input can also be specified as the externally guided or driven linear or angular coordinates corresponding to several joints (as many joints as there are degrees of freedom) on which mixed coordinates have been defined. This basic notion is explained by means of the following examples:7172 3. Kinematic AnalysisFigure 3.1. Four-bar mechanism modeledwith natural coordinates.Figure 3.2. Four-bar mechanism modeled with mixed coordinates.Example 3.1As a first example, the four-bar mechanism of Figure 3.1 will be considered. This sys-tem has four natural coordinates (x 1,y 1,x 2,y 2).The constraint equations corresponding to this mechanism are following constant distance conditions:x 1 Ð x A 2 + y 1 Ð y A 2 Ð L 22 =02 Ð x 12 + y 2Ð y Ð L 32 = 0x 2 Ð x B 2 + y 2 Ð y B 2 Ð L 42 = 0These three equations are not sufficient to determine the four unknown variables of the problem. In fact, it is still necessary to enter the condition that the position of the input element (element 2) is known. If both coordinates of point 1 are known, then only two unknown variables are left. In this case, it is obvious that the first constraint equation which establishes the constant length condition of element 2 no longer makes any sense, because it does not contain any unknown variable. Consequently the prob-lem reduces to the finding of x 2 and y 2, using the last two nonlinear quadratic con-straint conditions.Example 3.2Let us consider the four-bar mechanism shown in Figure 3.2, which uses mixed coor-dinates; that is, the same coordinates as in example 3.1 plus the angle y between ele-ments 2 and 3 at joint 1. Let's assume that, instead of the position of the input element,one knows the angle y . In this case the constraint equations will be as follows (assuming a suitable value for y to be able to use the scalar product):x 1 Ð x A 2 + y 1 Ð y A 2 Ð L 22 = 02 Ð x 12 + y 2 Ð y Ð L 32 = 0x 2 Ð x B 2 + y 2 Ð y B 2 Ð L 42 = 0x 1 Ð x A 2 Ð x 1 +1 Ð y y 2 Ð y Ð L 2 L 3 cos y = 03.1 Initial Position Problem 73Figure 3.3. RSCR mechanism modeled with natural coordinates.which is a system with four equations and four unknown variables, assuming that the externally driven angle y is known.Example 3.3Figure 3.3 depicts a three-dimensional four-bar mechanism RSCR (Revolute-Spherical-Cylindrical-Revolute) modeled with natural coordinates. This mechanism has three movable points and one movable unit vector; that is, twelve dependent Cartesian coordinates and one degree of freedom. Also the input angle y has been in-troduced as an additional externally driven coordinate. The constraint equations corre-sponding to this mechanism are the following:1. 1 Ð x 0 Ð x y 1 Ð y A y 0 Ð y A 1 Ð z 0 Ð z k 1 cos y = 02.1 Ð x 1 Ð y 1 Ð z Ð k2 = 03.1 Ð x Ax 1 Ð y Ay 1 Ð z Az Ð k 3 = 04.x 2 Ð x 2 Ð y 12 + z 2 Ð z Ð k 4 = 05.x 2 Ð x 1x + y 2 Ð y 1y + z 2 Ð z 1z Ð k 5 = 0 6.u 1x 2 + u 1y 2 + u 1z 2 Ð 1= 07.x 3 - x B + y 3 - y B + z 3 - z B Ð k 6 = 08.x 3 Ð x B u 1x 3 Ð y 1y + z 3 Ð z B u 1z Ð k 7 = 09.x 3 Ð x B u Bx + y 3 Ð y B u By + z 3 Ð z B u Bz Ð k 8 = 010.u Bx u 1x + u B y u 1y + u B z u 1z Ð k 9 = 011.y 3 Ð y 1z 3 Ð z 2 u 1y = 012.z 3 Ð z 1x Ð x 3 Ð x 1z = 013.x 3 Ð x 1y Ð y 3 Ð y 1x = 0This is the system of nonlinear equations that governs the position problem for the RSCR mechanism. The first equation corresponds to the input angle definition; equa-74 3. Kinematic Analysistions 2 and 3 represent rigid body condition for element 2; equations 4 to 6 represent rigid body constraints for element 3; equations 6 to 10 represent the same for element 4, and equations 11 to 13 (only two of them are independent) contribute to define the cylindrical joint. Finally, k i (i=1,...,9) represents constant values.The above examples clearly indicate that irrespective of the multibody sys-tems being considered, the position problem is always based on solving the con-straint equations, which make up the following set of equations:F(q, t)=0(3.1) where q is the vector of the system dependent coordinates. It will be assumed that there are at least as many equations as there are unknown variables or coor-dinates. To solve systems of nonlinear equations such as (3.1), it is customary to resort to the well-known Newton-Raphson method which has quadratic conver-gence in the neighborhood of the solution (the error in each iteration is propor-tional to the square of the error in the previous iteration) and does not usually cause serious difficulties if one starts with a good initial approximation.The Newton-Raphson method is based on a linearization of the system (3.1) and consists in replacing this system of equations with the first two terms of itsexpansion in a Taylor series around a certain approximation qi to the desiredsolution. Once the substitution has been made, the system (3.1) becomesF(q, t)@F(q i)+F q(q i)(q Ð q i)=0(3.2) where the time variable has not been accounted for, so that in this problem has a constant value. Matrix F q is the Jacobian matrix for constraint equations; that is to say, the matrix of partial derivatives of these equations with respect to the de-pendent coordinates. This matrix takes the following form:F q=¶f1¶q1¶f1¶q2.......¶f1¶q n¶f2¶q1¶f2¶q2.......¶f2¶q n ...................¶f m¶q1¶f m¶q2.......¶f m¶q n(3.3)In equation (3.3), m is the number of constraint equations and n the number of dependent coordinates. If the constraint equations are independent, f=n-m is the number of degrees of freedom of the multibody system.Equation (3.2) represents a system of linear equations constituting an approx-imation to the nonlinear system (3.1). The vector q, obtained from the solution of equation (3.2), will be an approximation of the solution in (3.1). By calling this approximate solution qi+1, a recursive formula is obtained as follows:F(q i)+F q(q i)(q i+1Ð q i)=0(3.4)3.1 Initial Position Problem 75qFigure 3.4. Iteration process of the Newton-Raphson method.which can be used repeatedly until the error in the system of equations (3.1) is insignificant, or until the difference between the results of two successive itera-tions is smaller than a pre-specified tolerance. Figure 3.4 shows the geometric representation of the Newton-Raphson method for the case of a nonlinear equa-tion with one unknown. The function F(q) is linearized at point qi , i.e. substi-tuted by its tangent linear space, which are the first two terms of the Taylor ex-pansion formula. The point where the tangent space intersects the horizontal axisis the approximate solution qi+1. The function F(q) is again replaced at pointq i+1by the new tangent space and a new approximate solution qi+2is found. Onearrives ultimately within the desired accuracy to the exact solution q.Note that the Newton-Raphson iteration will not always converge to a solu-tion. It has been pointed out that if the initial approximation is not close enough to a solution, the algorithm may diverge. There is still another source of difficul-ties. If the values of the input variables do not correspond to a possible physical solution, the mathematical algorithm will fail irrespective of how the initial ap-proximation has been chosen.The Jacobian matrix of the constraint equations, defined by means of equa-tion (3.3), plays an extremely important role in all kinematic and dynamic analy-sis problems. In the equation (3.4), the Jacobian matrix determines the linear equation system used to find the successive approximations for solving the ini-tial position problem. Evaluating and triangularizing this matrix easily and quickly are characteristics of all good multibody system analysis methods. The natural coordinates permit the performance of these operations in the best possible way.In Section 1.2, it was stated that the initial position problem had multiple so-lutions, and this is indeed the case. Depending on the vector qo where the itera-tion begins, some solution will be attained.Example 3.4To complete this section on the initial position problem, the equations (3.4) corre-sponding to the four-bar mechanism studied in Examples 3.1 and 3.2 will be com-76 3. Kinematic Analysisa)b)Figure 3.5. Iteration process of the Newton-Raphson method in a four-bar mechanism.pletely developed. The constraint equations of this case were presented in Example3.1, and consequently the equation (3.4) takes the following form:2x1Ðx A y1Ðy A00x1Ðx2y1Ðy2x2Ðx1y2Ðy100x2Ðx B y2Ðy B ix1y1x2y2i+1Ðx1y1x2y2i==Ðx1Ðx+1ÐyÐL22x2Ðx+2Ðy12ÐL32x2Ðx+2ÐyÐL42iIn this system of equations, at least one of the four unknown coordinates must be known ahead of time in order to be able to solve the problem. If, for example, x1 is known, then:(x1)i+1 Ð (x1)i = 0and the first column of the Jacobian matrix is multiplied by zero, meaning that it can be eliminated.In the case of the four-bar mechanism of Figure 3.2, modeled with mixed coordi-nates and whose constraint equations are presented in Example 3.2, equation (3.4) be-comes:2(x1Ð x A)2(y1Ð y A)0002(x1Ð x2)2(y1Ð y2)2(x2Ð x1)2(y2Ð y1)0002(x2Ð x B)2(y2Ð y B)0 (x2Ðx1+x AÐx1)(y2Ðy1+y AÐy1)(x1Ð x A)(y1Ð y A)(L2 L3 sin y)i..x1y1x2y2y i+1Ðx1y1x2y2y i=(x1Ðx A)2+(y1Ðy A)2Ð L22(x2Ðx1)2+(y2Ðy1)2Ð L32(x2Ðx B)2+(y2Ðy B)2Ð L42(x1Ðx A)(x2Ðx1)+(y1Ðy A)(y2Ðy1)Ð L2 L3 cos y i3.1 Initial Position Problem 77Figure 3.6. Possible divergence in the Newton-Raphson iterationUsually, the angle y is known; therefore, the last unknown variable (y i+1 Ð y i ) has a zero value. Thus the fifth column of the Jacobian matrix can be eliminated.One characteristic common to the Jacobians matrices shown in this example (and in all the Jacobians matrices calculated with natural coordinates) is that they are linear functions of the dependent variables. For example, Figures 3.5a and 3.5b include drawings of the initial approximation, the first iterations, and the final solution of the initial position problem in the two four-bar mechanisms of Figures 3.1 and 3.2 com-puted according to the above expressions.The Newton-Raphson method, explained in this section, converges rather quickly (quadratic convergence) when it is close to the desired solution. At times, and during the first iterations, it can give very abrupt jumps as a result of not having started from a sufficiently good initial approximation. Figure 3.6shows what could happen in this case. The approximate solution q i+1 is further away from the true solution q , than the previous approximation q i . It is even possible that the value of function F (q ), a function that should be equal to zero,could increase when moving from q i to q i+1.This problem is not easy to solve without resorting to much more compli-cated numerical methods. In general, one should do everything possible to start from good initial approximations. If this cannot be achieved, then one should try to apply a reduction to the coordinates modification given by equation (3.4) and to apply it to the previous approximate solution q i . As this often works, a cor-rection factor of 1/2 or 1/3 is recommended. Finally, one should always make sure that the module of F (q ) decreases when going from point q i to q i+1.Some authors have solved the position problem at times by calculating differ-ent solutions numerically by means of the so-called continuation methods (Tsai and Morgan (1985)). Continuation methods start out from a position where the multibody system complies with all the constraint equations, although the input elements might not be at the desired position and the fixed joints might not be at78 3. Kinematic Analysistheir final position. With relaxed conditions regarding the input elements and the fixed element, it is not difficult to find a position on the multibody system that satisfies the constraint equations. Then, by means of small finite displacements whose convergence is guaranteed by the Newton-Raphson method, an attempt is made to move the input elements and the fixed joints to their correct position. At times, the bifurcation points (points at which two or more possible movements can occur) provide a way of finding different solutions to the position problem.3.2 Velocity and Acceleration Analysis3.2.1 Velocity AnalysisThe equations that permit solving the velocity problem originate after one dif-ferentiates with respect to time the constraint equations. If these equations are represented symbolically asF (q , t ) = 0(3.5)by differentiating with respect to time, the following equation is obtained:F ,q =ÐF t ºb (3.6)where F q is the Jacobian matrix defined by means of equation (3.3). Vector q is the vector of dependent velocities (derivative with respect to the time of the vec-tor of dependent coordinates or position variables). Vector (ÐF t = b ) is the par-tial derivative of the constraint equations with respect to time. If all the con-straints are scleronomous, meaning that there are no rheonomous or time depen-dent constraints, this derivative will be zero) If the position of the multibody system is known, equation (3.6) allows us to determine the velocities of the multibody system by starting from the velocity of the input elements. Just as in the position problem, the matrix that controls the velocity problem is the Jacobian matrix of the constraint equations. The essential difference between both problems is that where the position problem is nonlinear, the equations governing the velocity problem are linear. This means that the equations do not have to be iterated, and there is only one solution to a properly posed problem.The following example illustrates these concepts:Example 3.5As an example of this, the velocity equations of the four-bar mechanism of Figure 3.1will be determined below by using: a) relative coordinates, b) reference point coordi-nates, c) natural coordinates, and d) mixed coordinates.a)Using relative coordinates , the constraint equations are given by (See Section2.1.1),L 1 cos Y 1 + L 2 cos (Y 1 + Y 2) + L 3 cos (Y 1 + Y 2 + Y 3) Ð O D = 03.2 Velocity and Acceleration Analysis 79L1 sin Y1+ L2 sin (Y1+Y2)+ L3 sin (Y1+Y2+Y3)=0Differentiating these equations with respect to time, we obtain:ÐL1 sin y1y1Ð L2 sin (y1+y2)(y1+y2)ÐÐ L3 sin (y1+y2+y3)(y1+y2+y3)=0L1 cos y1y1+ L2 cos (y1+y2)(y1+y2)++ L3 cos (y1+y2+y3)(y1+y2+y3)=0and by rearranging these equations, we arrive at:Ð L1s1Ð L2s12Ð L3s123Ð L2s12Ð L3s123Ð L3s123 L1c1+ L2c12+ L3c123 L2c12+ L3c123 L3c123y1 y2 y3where s1 = sin y1, s12 = sin (y1 + y2), and so forth.If one of the three velocities in the previous equation is known such as the one cor-responding to the input coordinate) the corresponding column of the Jacobian matrix can be moved to the right-hand side of the equation. This results in a system of two linear equations with two unknown velocities that can be solved with no difficulties.b)Using reference point coordinates,the constraint equations are represented by (SeeSection 2.1.2):x1ÐxÐL12 cos Y1=y1ÐyÐL12 sinY1=02Ðx1ÐL12 cos Y1Ð L22 cos Y2=y2ÐyÐL12 sin Y1Ð L22 sinY2=03Ðx2ÐL22 cos Y2Ð L32 cos Y3=y3ÐyÐL22 sin Y2Ð L32 sin Y3=0x3Ðx DÐL32 cos Y3=0y3Ðy DÐL32 sin Y3=0and the time derivatives are:x1+ L1/2Y1 sin Y1=0y1Ð L1/2Y1 cos Y1=0x2Ð x1+L12Y1 sin Y1+L22Y2 sin Y2=0y2Ð y1ÐL12Y1 cos Y1ÐL22Y2 cos Y2=0x3Ð x2+L22Y2 sin Y2+L32Y3 sin Y3=0y3Ð y2ÐL22Y2 cos Y2ÐL32Y3 cos Y3=0x3+L32y3 sin Y3=0y3ÐL32Y3 cos Y3=080 3. Kinematic AnalysisThese equations can be expressed in matrix form as follows:F q q =0where the matrix F q is10s1L1/200000001Ðc1L1/2000000Ð10s1L1/210s2L2/20000Ð1Ðc1L1/201Ðc2L2/2000000Ð10s2L2/210s3L3/20000Ð1Ðc2L2/201Ðc3L3/200000010s3L3/200000001Ðc3L3/2 which is a system of eight equations with nine unknown velocities. If the angular ve-locity y1 is known for element 2, the third column of the Jacobian matrix will be moved to the right side member. The result will be a system of eight linear equations with eight unknown velocities.c)With natural coordinates the constraint equations (Section 2.1.3) are representedby:(x1Ð x A)2+(y1Ð y A)2Ð L22=0(x2Ð x1)2+(y2Ð y1)2Ð L32=0(x3Ð x B)2+(y3Ð y B)2Ð L42=0whose time derivatives are:(x1Ð x A) x1+(y1Ð y A) y1=0(x2Ð x1)(x2Ð x1)+(y2Ð y1)(y2Ð y1)=0(x2Ð x B) x2+(y2Ð y B) y2=0and in matrix form yields:(x1Ðx A)(y1Ðy A)00 (x1Ðx2)(y1Ðy2)(x2Ðx1)(y2Ðy1) 00(x2Ðx B)(y2Ðy B)x1 y1 x2 y2By knowing one of the four natural velocities and by moving the corresponding column to the right-hand side of this equation, one can find the remaining velocities with the resulting set of three linear equations and three unknown variables.d)Using mixed coordinates, the constraint equations (Section 2.1.4) are:(x1Ð x A)2+(y1Ð y A)2Ð L22=0(x2Ð x1)2+(y2Ð y1)2Ð L32=0(x3Ð x B)2+(y3Ð y B)2Ð L42=0(x1Ð x A)(x2Ð x1)+(y1Ð y A)(y2Ð y1)Ð L2 L3 cos Y=03.2 Velocity and Acceleration Analysis 81Figure 3.7. Results of a velocity analysis in a four-bar mechanism.differentiating with respect to time:x1Ðx A x1+y1Ðy A y1=0x2Ðx x2Ðx+y2Ðy y2Ðy=0x2Ðx B x2+y2Ðy B y2=02Ðx1 x1+x1Ðx AÐx1+2Ðy1 y1++y1Ðy A y2Ðy1+L2L3 sin y y=0which can be expressed in matrix form as1Ðx1Ðy0001Ðx21Ðy22Ðx12Ðy10002Ðx2Ðy0x2Ð2x1+x A y2Ð2y1+y A1Ðx1Ðy L2L3sin yx1y1x2y2y=If y is known, the fifth column will be moved to the right-hand side and will leave four equations with four unknowns. Figure 3.7 shows the result of a velocity analysis in accordance with an input velocity of y =1.3.2.2 Acceleration AnalysisThe finding of the dependent acceleration vector q becomes apparent by simply differentiating with respect to time the velocity equation (3.6). This yields the following result:F q,q=ÐtÐq q º c(3.7)If the position vector q and the velocity vector q are known, by solving the system of linear equations (3.7), one can find the dependent acceleration vector q. Note that the leading matrix of the systems of linear equations (3.6) and (3.7) is exactly the same. As a consequence, if it has been formed and triangularized82 3. Kinematic AnalysisFigure 3.8. Results of an acceleration analysis in a four-bar mechanism.to solve the velocity problem, the acceleration analysis can be carried out by simply forming the right-hand side and by performing a forward reduction and backward substitution. When there are no rheonomous or time-dependent con-straints, the velocity problem is homogeneous; whereas the acceleration problem is always non-homogeneous as long as the velocities are not equal to zero.Equation (3.7) can be differentiated once again to obtain the jerk or over ac-celeration equation:F q d dt = Ð t Ð 2 q q Ð q q (3.8)Once again a system of linear equations has been obtained whose leadingmatrix is the Jacobian matrix of the constraint equations.Example 3.6Included below are the acceleration equations for the four-bar mechanism of Example3.5d modeled with mixed coordinates. These equations are obtained by differentiating the corresponding velocity equations:1Ðx 1Ðy 0001Ðx 21Ðy 22Ðx 12Ðy 10002Ðx 2Ðy 0x 2Ð2x 1+x A y 2Ð2y 1+y A1Ðx 1Ðy L 2 L 3 sin y = Ð x 1y 1000x 1Ðx y 1Ðy x 2Ðx y 2Ðy 000x 2y 20x 2Ð2x 1y 2Ð21x 1y 1L 2 L 3 cos y x 1y 1x 2y 2y3.2 Velocity and Acceleration Analysis 83If all the velocities q and input accelerations y are known, the remaining accelera-tions q can be calculated by means of the four equations with four unknowns resulting from moving the fifth column, multiplied by y, to the RHS of the acceleration equa-tion. Figure 3.8 graphically shows the result of an acceleration analysis that corre-sponds to the expression developed in this example.3.3 Finite Displacement AnalysisThe finite displacement analysis is closely related to the initial position problem, and is controlled by the same system of nonlinear equations (the kinematic constraint equations). The velocity and acceleration analyses are used at times in finite displacement analysis to improve the initial approximation with which the iterative process begin, which explains the reason for including it here and not immediately after the initial position problem.3.3.1 Newton-Raphson IterationAs explained in Section 1.2, once one knows a position of the multibody system where all the constraint equations are satisfied, the finite displacement problem consists of finding the new position that the system takes when a finite displace-ment is applied to each one of the input elements or externally driven relative coordinates. Finite displacement is understood to be any movement other than infinitesimal.The main problem dealt with in this section is of the same nature and conse-quently controlled by the same equations of the position problem. Therefore, the Newton-Raphson method can be used for solving it. The difference between both problems lies in the fact that the finite displacement problem usually relies on a good initial approximation which is obtained from a previous exact posi-tion where all the elements satisfy the constraints. It is possible to improve upon the approximation by means of a velocity and acceleration analysis, as will be described in the next section.These advantages do away with many of the convergence problems encoun-tered in the initial position problem. In addition, the problem of multiple solu-tions becomes marginal. If the displacement of the input elements is small enough, then of all the possible solutions for the constraint equations, the correct one will be the closest to the starting position. This is precisely the one obtained by the Newton-Raphson iterations. However, there still remains the possibility of driving or trying to drive the multibody system to unfeasible positions, that is, positions that cannot be reached without violating some constraints equations. Trying to move the end-effector of a robot out of its workspace is an example of a finite displacement problem where the Newton-Raphson method will necessar-ily fail to find a correct solution.84 3. Kinematic AnalysisBFigure 3.9. Improving the initial approximation.A2' BFigure 3.10. Better estimate for an initial approximation.3.3.2 Improved Initial ApproximationIn order to determine and improve the initial or starting approximation, the ex-ample of the four-bar mechanism will be used once again. This will clearly de-scribe the method without any loss of generality.Figure 3.9 shows a four-bar mechanism, in which the input element has been rotated a finite angle. One possible way of generating an initial approximation is by not varying the remaining natural coordinates as in the starting position shown in Figure 3.9. This approximation leads to a severe violation of the con-straint equations.The initial approximation shown in Figure 3.9 can be improved upon by means of velocity analysis, as indicated in Figure 3.10. The velocity analysis is carried out by imposing a velocity at the input element so that the endpoint 1' of the velocity vector of 1 is the closest point to 1" over the perpendicular to A-1 (1'-1" is parallel to A-1).3.3 Finite Displacement Analysis 85Since 1" is known, it is not difficult to determine the velocity of the input el-ement such that the end of the velocity vector at point 1 is 1'. Using this velocity as input, a velocity analysis is performed, and the ends of the velocity vectors are determined for all the basic points of the mechanism (in Figure 3.10, 2' is the end of the velocity vector of point 2).The initial approximation used to start the iterations for the Newton-Raphson method is indicated by the dotted lines in Figure 3.10 It is an improvement over the one in Figure 3.9. Note that the initial approximation is (A-1"-2'-B) and not (A-1'-2'-B). The exact position 1" of point 1 is known because it belongs to the input element and this exact position should be used.It is not essential that point 1' be the closest one to 1" on the tangent to the trajectory of 1. Another simpler possibility for calculating point 1' and the velocity of the input element, is to assume that point 1 changes to position 1" in an arbitrary period of time such as 1 second. Next, calculate the angular velocity of the input element by dividing angle 1-1" (in radians) by the said amount of time where the quotient is the said angular velocity. The position at the initial approximation of any point P can be calculated by means of the following expression:q = q o + q D t (3.9) Equation (3.9) is an approximate integral of velocities starting from the previ-ous position. An approximate integration which also causes the accelerations to intervene can be obtained in a similar manner:q D t2(3.10)q = q o + q D t + 12This formula suggests that the initial approximation can be constructed start-ing from a velocity analysis and an acceleration analysis. To calculate the veloc-ity and acceleration of the input element one may proceed as follows:1.Apply one of the previously studied methods and determine the velocity ofthe input elements.2.Knowing the initial and final position of the input elements and their veloc-ity, determine the acceleration to be applied to them applying equation (3.10) to the input elementsDetermination of the initial approximation by means of velocity and accelera-tion analysis allows the iterations to begin with a better approximation to the fi-nal solution. The cost of an acceleration analysis is small if a velocity analysis has already been performed. The matrix for both systems of equations is the same, and one only needs to form it and triangularize it once. Based on the expe-rience gained through numerical experiments performed by the authors, the ini-tial approximation constructed with velocities and accelerations does not always give better results than the one determined from velocities only.。

asymptotic analysis缩写

asymptotic analysis缩写
Asymptotic Analysis 的意思是 "极限分析",指的是在算法或
数学模型中,当样本数量趋近于无穷大时,所计算的结果或估计值趋
向于某个确定的极限值,通常称为无穷大极限或无穷级数。

这种分析方法常用于对连续函数、概率分布等进行分析和估计。

例如,在数学中,当函数 $f(x)$ 在 $x=a$ 处取得极小值时,可
以通过引入 $a$ 的无穷大来估计 $f(x)$ 的值。

这个估计值可以表示为 $f(a) approx lim_{x to a^-} f(x)$。

其中,$lim_{x to a^-} f(x)$ 表示当 $x$ 趋近于 $a$ 时,$f(x)$ 的值趋近于某个值,这个值称为极限值。

Asymptotic Analysis 是一种重要的数学分析方法,可以帮助研究者更好地理解并预测复杂系统的性能和行为,尤其是在当样本数量趋近于无穷大时,系统的行为可能会表现出非常惊人的规律和特性。

往复泵曲柄滑块机构的运动学分析

往复泵曲柄滑块机构的运动学分析付子豪，翟国栋(中国矿业大学(北京）机电与信息工程学院，北京100083)摘要:基于往复式泵的结构及工作原理，文中用解析法对往复泵中的曲柄滑块机构滑块的运动规律进行了分析与讨论，其中对滑块的速度和加速度进行了定量表征，并建立了曲柄滑块的运动本构方程。

对于曲柄的特定位置，以C语言程序编程对滑块的相关特征参数进行了计算。

这些结果表明：文中探索的曲柄滑块机构运动学分析研究方法具有计算精度高、分析方便、通用性强的特点。

因此，该研究对实际曲柄滑块机构的逆向设计具有重要的参考价值和指导意义。

关键词:往复式抽水泵；曲柄滑块;运动分析中图分类号:TH 133 文献标志码:A文章编号：1002-2333(2016)12-0119-04 Kinematics Analysis on the Crank Slider of Reciprocating PumpFU Zihao，ZHAI Guodong(School of Electrical and Information Engineering, China University of Mining and Technology (Beijing), Beijing 100083, China) Abstract：Based on the structural characterizations and working principle of reciprocating pump, the analytical method is used to analyze the slider motion low of crank -slider mechanism in reciprocating pump, in which the velocity and acceleration of the slider are quantitatively characterized. This paper establishes the constitutive equation of motion of slider crank mechanism. For the specific position of the crank, the related characteristic parameters of the sliding block are calculated using the C programming language program. These results indicate that this method has the advantages of high accuracy, simple and strong commonality. Therefore, this study has important reference value and guiding significance for the design of the actual crank slider mechanism.Key words：reciprocating pump; crank slider; kinematic analysis0引言在往复式泵的结构设计中，往往需要对其主体机构曲柄滑块机构进行运动学及强度分析，并且根据运动学分析结果建立起相应的数学模型。

1-D KINEMATICS - LESSON 1(加拿大高中物理第一课)

goal should be to become very familiar with their meaning. You may
click on any word now to
Kinematics is the science of describing the motion of objects using words, diagrams, numbers, graphs, and euations. Kinematics is a branch of mechanics. The goal of any study of kinematics is to develop sophisticated mental models which serve to describe (and ultimately, explain) the motion of real-world objects.
investigation into the physics of motion. As we focus on the language, principles, and laws which describe and explain the motion of objects, your efforts should center around internalizing the meaning of the information. Avoid memorizing the information; and avoid abstracting the information from the physical world which it describes and explains. Rather, contemplate the information, thinking about its meaning and its applications.

机械工程专业英语

【The branch of scientific analysis which deals with motions time and forces is called mechanics and is made up of two parts statics and dynamics】. 对运动、时间和作用力进行科学分析的分支称为力学，它由静力学和动力学俩部分组成。

【Statics deals with the analysis of Stationary ,i .e.,those in which time is not a factor,and dynamics deals with systems which change with time 静力学对静止系统进行分析，即在其中不考虑时间这个因素，动力学对随时间而变化的系统进行分析。

【Forces are transmitted into machine members through matingsurfaces ,e.g.,form a gear to a shaft or form one gear through meshing teeth to another gear,from a V belt to a pulley,or from a cam to a follower】.力通过接触表面传到机器中的各构件上。

例如，从齿轮传到轴或者从齿轮通过与其啮合的轮齿传到另一个齿轮，从V带传到带轮，或者从凸轮传到从动件。

【It is necessary to know the magnitudes of these forces for a variety of Reasons】由于很多原因，人们必须知道这些力的大小。

【The distribution of the forces at the boundaries or mating surfaces must be reasonable and their intensities must be within the working limits of the materials composing thesurfaces】这些力在边界或在接触表面的分布必须合理，它们的大小必须在构成配合表面的材料的工作极限以内。

电气自动化专业英语单词(按首字母排列)

电气自动化专业英语单词（按首字母排列）abbreviate缩写，缩写为abciaa某i横坐标aboluteencoder绝对编码器acmotor交流环电动机acquirrelcageinductionmotoracademic纯理论的active(paive)circuitelementactiveinrepectto相对…呈阻性activeregion动态区域active有源的actuate激励，驱动actuator执行机构actuator执行器adjacent临近的,接近的adjacent相邻的，邻近的Adjutable-voltageinverter电压型逆变器admittance导纳advent 出现airgap气隙aircraft飞机air-gapflu某ditribution气隙磁通分布air-gapflu某气隙磁通air-gapline气隙磁化线algebraic代数的algebraic代数的algebraical代数的algorithm算法algorithmic算法的align调整，校准allowabletemperaturerie允许温升alloy合金allude暗指，直接提到alnico铝镍钴合金alphabet字母表alternatingcurrent,AC交流aluminum铝ambient环境的ambiguity 模棱两可Ammeter安培计电流表ammeter电流表ampere-turn安匝(数)amplidyne微场扩流发电机amplification扩大AmplitudeModulation(AM调幅analogelectronic电力电子学analog-to-digitalconverion,ADC模数转换器analytical分析的，分解的analytical解析的angular角的anode阳极，正极antenna天线aptly适当地，适宜地arbitration仲裁，公断arcwelding电弧焊armaturecircuit电枢电路armaturecoil电枢线圈armaturemmfwave电枢磁势波armature电枢armature电枢armature 衔铁arrangement结构aaruleofthumb根据经验aynchronoumachine异步电机attenuate衰减audio音频的automaticocillograph自动示波器automatictation无人值守电站automaticVoltageregulator(AVR)automobiletartermotor汽车启动机automobile汽车autonomic自治的autonomou匿名的autotranformer自耦变压器au某iliarymotor辅助电动机au某iliary辅助的au某iliary辅助的backlah啮合间隙，齿隙ballat镇流器bandwidth带宽barcodereader条码阅读器bae基极bearing轴承bearing轴承bellow膜盒bilateralcircuit双向电路bimotored双马达的binary二进制binary-codeddecimal,BCDbiphae双相的bipolarjunctiontranitor(BJT双极性晶体管bitablecircuit双稳电路blend混合，调和，配料blockdiagram方框图blow（保险丝）烧断bodeplot波特图bolt螺栓boot增压boot-buck升压去磁boredom讨厌，无趣braking制动branchcircuit直路breakawayforce起步阻力breakdowntorque极限转矩breakdown击穿bronze青铜bruh电刷brute僵化的buck补偿buhing高压套buhing套管bypa旁路by-product副产品calibrate校正calibration标定，标准化calibration校准，标定，刻度callfor 需要cam凸轮cantilever悬臂capability容量capacitanceeffect电容效应capacitor电容器capacitor电容器capacity容量capule封装carbon碳carbon-filamentlamp碳丝灯泡carrier载波Carteiancoordinate笛卡儿坐标系cartridge盒式保险丝centrifugalforce离心力centrifugal离心的，离心力ceramic陶瓷的chamber室，腔chao混乱checkum检查和choppercircuit斩波电路circuitbranch支路circuitparameter电路参数circuitry电路，线路circumference 圆周circumnavigate饶过clamp夹，钳clamp夹住，夹紧claic古典的，经典的，传统的clearance间隙client-ervermodel客户服务器模型client-erver客户-服务器clinker-cooler熟料冷却器cloed-loop闭环■coat跟踪惯性coa某ial共轴的,同轴的cogging齿槽效应coilwinding线圈绕组coil线圈绕组coincideinphaewith与…同相coincidence一致，相等collector 集电极collector集电极]condominium（国际）共官conductance电导conductor导体conduit导线，导线管conepulley塔轮，快慢轮configuration组态connection接线端contraint强制，约束contact触点contactor接触器contiguou邻近的conveyance运输工具conveyor传送机copperbar铜导条copperendring铜端环core铁心corona电晕,放电corridor通道，走廊corridor通路corroion腐蚀cot-effective花费大的counterelectromotiveforce，CEMF反电势counteremf反电势counteract抵抗，抵消，消除counterclockwie逆时针counterpart对应物couplingcapacitor结合电容creep蠕动criteria标准，判据crude不精细的，粗略的crytal晶体crytal晶体的，水晶，晶体cubicle立方体culminate达到极值点culprit犯罪者CyclicRedundancyCheck循环冗余检查cylindrical圆柱式的damper减速器dahpotrelay油壶式继电器dahpot阻尼器dcgenerator直流发电机DClink直流环节dcmotor直流电动机demachine直流电机decouple解耦，去除干扰deenergize不给…通电deflection挠度，挠曲demagnetization退磁，去磁demodulation解调demodulator解调器demytify阐明denominator分母depict描绘，描写depict描述depre压下derivative导数derive推倒deteriorate使…恶化deterioration变化，降低品质deterioration损坏,磨损deviation 偏差dial刻度盘dial刻度盘，调节控制盘diameter直径diameter直径diaphragm膜，隔板diaphragm膜片diaphragm膜片，挡板diaphragm震动膜dictate确定differentialpreuretranducer差压变送器differentiation微分differentiation微分diode二极管diplacementcurrent位移电流diplacement位移diipate浪费diipate散发ditillation蒸馏ditributedytem分布式系统ditribution分配，配电doublye某cited双边励磁drill钻床due应得到的dungen地牢dwelling住房dynamicbraking能耗制动dynamicrepone动态响应dynamic-tateoperation动态运行dynamometer测力计，功率计eddycurrentbraking涡流制动eddycurrent涡流eddycurrent涡流eddy 涡流effectivevalue有效值effectofaturation饱和效应elape过去，消逝elape时间（流逝）elbow弯头electricenergy电能electricaldevice电气设备electricaltreing电气应力electrode 电极电焊条electrodynamometer电测力计electro-hydraulic电动液压的electrolytic电解的electromagneticinterference电磁干扰electromagnetictorque电磁转矩electromechanical机电的electronicmail电子邮件electro-pneumatic电动气动的eluive难以捉摸的emf=electromotivefore电动势emitter发射管放射器发射极emitter发射极encloure机壳encloure设备外壳encloure外（机）壳encode编码encoder编码器endring端环energize励磁energyconverter电能转换器entity实体enumerate列举enviion预见epochangle初相角equilibria平衡equilibriumlevel平均值equivalentcircuit等效电路equivalentT–circuitT型等值电路errordetector误差检测器errorignal误差信号error误差，偏差ecalation升级，提高etablihment组织，部门etiquette规则e某citationytem励磁系统e某citedby励磁e某citingvoltage励磁电压e某pedite加速e某pel排出，放出e 某pire期满,终止e某ponential指数e某ternalarmaturecircuit电枢外电路e某ternalcharacteritic 外特性e某truded型材的fabricate制造faithful正确的，可靠的fallout余波，附带结果faten固定，连接feaible可行的feeder馈电线，电源线，馈电板feedforward前馈felt毡ferromagnetic铁磁的fidelity保真度fidelity重现精度，真实，正确fieldcoil励磁线圈fieldcurrent 励磁电流fieldeffecttranitor(FET)场效应管fieldpole磁极fieldwinding磁场绕组励磁绕组fieldwinding励磁绕组figureofmerit品质因数，优值filter滤波器fin飞边fi某ture设备，装置]flicker闪烁，摇曳flip-flop触发器fluctuation升降剥动，不规则的变化fluorecent荧光的，有荧光性的flu某denity磁通密度flu某linkage磁链flu某perpole每极磁通form-wound模绕forwardtranferfunction正向传递函数forward转发fraction分数frame机座，机壳frequency-domain频域FrequencyShiftKeying(FSK)移频键控frequency频率friction摩擦fullload满载full-duple某全双工full-loadtorque满载转矩furnace炉fue保险丝，熔丝fue熔断器fue熔断器，保险丝gain增益gain增益gamut全体，整体gear齿轮，传动装置general-purpoerelay通用继电器generating发电generatorvoltage发电机电压generator发电机generator发电机Geometricalpoition几何位置geometry几何结构glitch同步glue胶合，粘贴goggle护目镜，潜水镜graphite石墨grinder磨床groly大概，大体上的ground-faultcircuitinterrupter（GFCI）ground-faultprotector （GFP）gyrocope陀螺仪half-duple某半双工hand-wheel手轮，驾驶盘，操纵盘hardwired硬接线的harmonic谐波的havoc大破坏hazard危险hazardou危险的heatink散热器heatingappliance电热器hierarchy阶梯，等级high-gain高增益high-pafilter高通滤波器high-performance高性能的high-volume 大容量hitherto迄今,至今hockeypuck冰球hoit起重机horepower马力horepower马力horehoemagnet马蹄形磁铁hot主机humidity湿度hydraulic液力的hydraulic液压传动hydraulic液压的，液压传动装置hydropowertation水电站hyterei 磁滞接地故障保护器，接地故障断路器idealource理想电源ideological思想的imaginarypart虚部immunity抗扰性impedance阻抗impedance阻抗impedance阻抗impule推动力in（inch,inche）英寸inparallelwith并联ineriewith串联intermof根据，在……方面inthevicinityof在…附近，在…左右incident入射的incrementencoder增量编码器indicatingneedle仪表指针indipenable必需的，必不可少的inducedcurrent感生电流induced-draftfan吸风机inductioncoupling感应耦合inductiongenerator感应发电机inductionmachine感应电机inductionmachine感应式电机inductionmotor感应电动机inductionmotor感应电动机inertial惯性的，惯量的inference干扰infinitevoltagegain无穷大电压增益infratructure基础，底层结构inherent固有的inhibit禁止initiate引起，促进injectionmolding注模inruhcurrent涌流intantaneouelectricpower瞬时电功率intantaneoumechanicalpower瞬时机械功率intructionet指令集inulation绝缘inulation绝缘inulation绝缘inulatortring绝缘子串intake吸入integrate求…的积分integratedcircuit集成电路integration积分integration积分下限interactive交互式interconnection相互连接interfacedataunit接口数据单元interface接口interferewith有害于。

机械设计专业术语的英语翻译

如有你有帮助，请购买下载，谢谢！阿基米德蜗杆 Archimedes worm安全系数 safety factor; factor of safety安全载荷 safe load凹面、凹度 concavity扳手 wrench板簧 flat leaf spring半圆键 woodruff key变形 deformation摆杆 oscillating bar摆动从动件 oscillating follower摆动从动件凸轮机构 cam with oscillating follower 摆动导杆机构 oscillating guide-bar mechanism摆线齿轮 cycloidal gear摆线齿形 cycloidal tooth profile摆线运动规律 cycloidal motion摆线针轮 cycloidal-pin wheel包角 angle of contact保持架 cage背对背安装 back-to-back arrangement背锥 back cone ；normal cone背锥角 back angle背锥距 back cone distance比例尺 scale比热容 specific heat capacity闭式链 closed kinematic chain闭链机构 closed chain mechanism臂部 arm变频器 frequency converters变频调速 frequency control of motor speed变速 speed change变速齿轮 change gear ; change wheel变位齿轮 modified gear变位系数 modification coefficient标准齿轮 standard gear标准直齿轮 standard spur gear表面质量系数 superficial mass factor表面传热系数 surface coefficient of heat transfer 表面粗糙度 surface roughness并联式组合 combination in parallel并联机构 parallel mechanism并联组合机构 parallel combined mechanism并行工程 concurrent engineering并行设计 concurred design, CD不平衡相位 phase angle of unbalance不平衡 imbalance (or unbalance)不平衡量 amount of unbalance 不完全齿轮机构 intermittent gearing波发生器 wave generator波数 number of waves补偿 compensation参数化设计 parameterization design, PD残余应力 residual stress操纵及控制装置 operation control device槽轮 Geneva wheel槽轮机构 Geneva mechanism ；Maltese cross槽数 Geneva numerate槽凸轮 groove cam侧隙 backlash差动轮系 differential gear train差动螺旋机构 differential screw mechanism差速器 differential常用机构 conventional mechanism; mechanism in common use 车床 lathe承载量系数 bearing capacity factor承载能力 bearing capacity成对安装 paired mounting尺寸系列 dimension series齿槽 tooth space齿槽宽 spacewidth齿侧间隙 backlash齿顶高 addendum齿顶圆 addendum circle齿根高 dedendum齿根圆 dedendum circle齿厚 tooth thickness齿距 circular pitch齿宽 face width齿廓 tooth profile齿廓曲线 tooth curve齿轮 gear齿轮变速箱 speed-changing gear boxes齿轮齿条机构 pinion and rack齿轮插刀 pinion cutter; pinion-shaped shaper cutter齿轮滚刀 hob ,hobbing cutter齿轮机构 gear齿轮轮坯 blank齿轮传动系 pinion unit齿轮联轴器 gear coupling齿条传动 rack gear齿数 tooth number齿数比 gear ratio齿条 rack如有你有帮助，请购买下载，谢谢！齿条插刀 rack cutter; rack-shaped shaper cutter齿形链、无声链 silent chain齿形系数 form factor齿式棘轮机构 tooth ratchet mechanism插齿机 gear shaper重合点 coincident points重合度 contact ratio冲床 punch传动比 transmission ratio, speed ratio传动装置 gearing; transmission gear传动系统 driven system传动角 transmission angle传动轴 transmission shaft串联式组合 combination in series串联式组合机构 series combined mechanism串级调速 cascade speed control创新 innovation ; creation创新设计 creation design垂直载荷、法向载荷 normal load唇形橡胶密封 lip rubber seal磁流体轴承 magnetic fluid bearing从动带轮 driven pulley从动件 driven link, follower从动件平底宽度 width of flat-face从动件停歇 follower dwell从动件运动规律 follower motion从动轮 driven gear粗线 bold line粗牙螺纹 coarse thread大齿轮 gear wheel打包机 packer打滑 slipping带传动 belt driving带轮 belt pulley带式制动器 band brake单列轴承 single row bearing单向推力轴承 single-direction thrust bearing单万向联轴节 single universal joint单位矢量 unit vector当量齿轮 equivalent spur gear; virtual gear当量齿数 equivalent teeth number; virtual number of teeth 当量摩擦系数 equivalent coefficient of friction当量载荷 equivalent load刀具 cutter导数 derivative倒角 chamfer 导热性 conduction of heat导程 lead导程角 lead angle等加等减速运动规律 parabolic motion; constant acceleration and deceleration motion等速运动规律 uniform motion; constant velocity motion等径凸轮 conjugate yoke radial cam等宽凸轮 constant-breadth cam等效构件 equivalent link等效力 equivalent force等效力矩 equivalent moment of force等效量 equivalent等效质量 equivalent mass等效转动惯量 equivalent moment of inertia等效动力学模型 dynamically equivalent model底座 chassis低副 lower pair点划线 chain dotted line（疲劳）点蚀 pitting垫圈 gasket垫片密封 gasket seal碟形弹簧 belleville spring顶隙 bottom clearance定轴轮系 ordinary gear train; gear train with fixed axes动力学 dynamics动密封 kinematical seal动能 dynamic energy动力粘度 dynamic viscosity动力润滑 dynamic lubrication动平衡 dynamic balance动平衡机 dynamic balancing machine动态特性 dynamic characteristics动态分析设计 dynamic analysis design动压力 dynamic reaction动载荷 dynamic load端面 transverse plane端面参数 transverse parameters端面齿距 transverse circular pitch端面齿廓 transverse tooth profile端面重合度 transverse contact ratio端面模数 transverse module端面压力角 transverse pressure angle锻造 forge对称循环应力 symmetry circulating stress对心滚子从动件 radial (or in-line ) roller follower对心直动从动件 radial (or in-line ) translating follower如有你有帮助，请购买下载，谢谢！对心移动从动件 radial reciprocating follower对心曲柄滑块机构 in-line slider-crank (or crank-slider) mechanism 多列轴承 multi-row bearing多楔带 poly V-belt多项式运动规律 polynomial motion多质量转子 rotor with several masses惰轮 idle gear额定寿命 rating life额定载荷 load ratingII 级杆组 dyad发生线 generating line发生面 generating plane法面 normal plane法面参数 normal parameters法面齿距 normal circular pitch法面模数 normal module法面压力角 normal pressure angle法向齿距 normal pitch法向齿廓 normal tooth profile法向直廓蜗杆 straight sided normal worm法向力 normal force反馈式组合 feedback combining反向运动学 inverse ( or backward) kinematics反转法 kinematic inversion反正切 Arctan范成法 generating cutting仿形法 form cutting方案设计、概念设计 concept design, CD防振装置 shockproof device飞轮 flywheel飞轮矩 moment of flywheel非标准齿轮 nonstandard gear非接触式密封 non-contact seal非周期性速度波动 aperiodic speed fluctuation非圆齿轮 non-circular gear粉末合金 powder metallurgy分度线 reference line; standard pitch line分度圆 reference circle; standard (cutting) pitch circle分度圆柱导程角 lead angle at reference cylinder分度圆柱螺旋角 helix angle at reference cylinder分母 denominator分子 numerator分度圆锥 reference cone; standard pitch cone分析法 analytical method封闭差动轮系 planetary differential复合铰链 compound hinge 复合式组合 compound combining复合轮系 compound (or combined) gear train 复合平带 compound flat belt复合应力 combined stress复式螺旋机构 Compound screw mechanism 复杂机构 complex mechanism杆组 Assur group干涉 interference刚度系数 stiffness coefficient刚轮 rigid circular spline钢丝软轴 wire soft shaft刚体导引机构 body guidance mechanism刚性冲击 rigid impulse (shock)刚性转子 rigid rotor刚性轴承 rigid bearing刚性联轴器 rigid coupling高度系列 height series高速带 high speed belt高副 higher pair格拉晓夫定理 Grashoff`s law根切 undercutting公称直径 nominal diameter高度系列 height series功 work工况系数 application factor工艺设计 technological design工作循环图 working cycle diagram工作机构 operation mechanism工作载荷 external loads工作空间 working space工作应力 working stress工作阻力 effective resistance工作阻力矩 effective resistance moment公法线 common normal line公共约束 general constraint公制齿轮 metric gears功率 power功能分析设计 function analyses design共轭齿廓 conjugate profiles共轭凸轮 conjugate cam构件 link鼓风机 blower固定构件 fixed link; frame固体润滑剂 solid lubricant关节型操作器 jointed manipulator惯性力 inertia force如有你有帮助，请购买下载，谢谢！惯性力矩 moment of inertia ,shaking moment惯性力平衡 balance of shaking force惯性力完全平衡 full balance of shaking force惯性力部分平衡 partial balance of shaking force 惯性主矩 resultant moment of inertia惯性主失 resultant vector of inertia冠轮 crown gear广义机构 generation mechanism广义坐标 generalized coordinate轨迹生成 path generation轨迹发生器 path generator滚刀 hob滚道 raceway滚动体 rolling element滚动轴承 rolling bearing滚动轴承代号 rolling bearing identification code 滚针 needle roller滚针轴承 needle roller bearing滚子 roller滚子轴承 roller bearing滚子半径 radius of roller滚子从动件 roller follower滚子链 roller chain滚子链联轴器 double roller chain coupling滚珠丝杆 ball screw滚柱式单向超越离合器 roller clutch过度切割 undercutting函数发生器 function generator函数生成 function generation含油轴承 oil bearing耗油量 oil consumption耗油量系数 oil consumption factor赫兹公式 H. Hertz equation合成弯矩 resultant bending moment合力 resultant force合力矩 resultant moment of force黑箱 black box横坐标 abscissa互换性齿轮 interchangeable gears花键 spline滑键、导键 feather key滑动轴承 sliding bearing滑动率 sliding ratio滑块 slider环面蜗杆 toroid helicoids worm环形弹簧 annular spring 缓冲装置 shocks; shock-absorber灰铸铁 grey cast iron回程 return回转体平衡 balance of rotors混合轮系 compound gear train积分 integrate机电一体化系统设计 mechanical-electrical integration system design 机构 mechanism机构分析 analysis of mechanism机构平衡 balance of mechanism机构学 mechanism机构运动设计 kinematic design of mechanism机构运动简图 kinematic sketch of mechanism机构综合 synthesis of mechanism机构组成 constitution of mechanism机架 frame, fixed link机架变换 kinematic inversion机器 machine机器人 robot机器人操作器 manipulator机器人学 robotics技术过程 technique process技术经济评价 technical and economic evaluation技术系统 technique system机械 machinery机械创新设计 mechanical creation design, MCD机械系统设计 mechanical system design, MSD机械动力分析 dynamic analysis of machinery机械动力设计 dynamic design of machinery机械动力学 dynamics of machinery机械的现代设计 modern machine design机械系统 mechanical system机械利益 mechanical advantage机械平衡 balance of machinery机械手 manipulator机械设计 machine design; mechanical design机械特性 mechanical behavior机械调速 mechanical speed governors机械效率 mechanical efficiency机械原理 theory of machines and mechanisms机械运转不均匀系数 coefficient of speed fluctuation机械无级变速 mechanical stepless speed changes基础机构 fundamental mechanism基本额定寿命 basic rating life基于实例设计 case-based design,CBD基圆 base circle如有你有帮助，请购买下载，谢谢！基圆半径 radius of base circle基圆齿距 base pitch基圆压力角 pressure angle of base circle基圆柱 base cylinder基圆锥 base cone急回机构 quick-return mechanism急回特性 quick-return characteristics急回系数 advance-to return-time ratio急回运动 quick-return motion棘轮 ratchet棘轮机构 ratchet mechanism棘爪 pawl极限位置 extreme (or limiting) position极位夹角 crank angle between extreme (or limiting) positions计算机辅助设计 computer aided design, CAD计算机辅助制造 computer aided manufacturing, CAM计算机集成制造系统 computer integrated manufacturing system, CIMS计算力矩 factored moment; calculation moment计算弯矩 calculated bending moment加权系数 weighting efficient加速度 acceleration加速度分析 acceleration analysis加速度曲线 acceleration diagram尖点 pointing; cusp尖底从动件 knife-edge follower间隙 backlash间歇运动机构 intermittent motion mechanism减速比 reduction ratio减速齿轮、减速装置 reduction gear减速器 speed reducer减摩性 anti-friction quality渐开螺旋面 involute helicoid渐开线 involute渐开线齿廓 involute profile渐开线齿轮 involute gear渐开线发生线 generating line of involute渐开线方程 involute equation渐开线函数 involute function渐开线蜗杆 involute worm渐开线压力角 pressure angle of involute渐开线花键 involute spline简谐运动 simple harmonic motion键 key键槽 keyway交变应力 repeated stress 交变载荷 repeated fluctuating load交叉带传动 cross-belt drive交错轴斜齿轮 crossed helical gears胶合 scoring角加速度 angular acceleration角速度 angular velocity角速比 angular velocity ratio角接触球轴承 angular contact ball bearing角接触推力轴承 angular contact thrust bearing角接触向心轴承 angular contact radial bearing角接触轴承 angular contact bearing铰链、枢纽 hinge校正平面 correcting plane接触应力 contact stress接触式密封 contact seal阶梯轴 multi-diameter shaft结构 structure结构设计 structural design截面 section节点 pitch point节距 circular pitch; pitch of teeth节线 pitch line节圆 pitch circle节圆齿厚 thickness on pitch circle节圆直径 pitch diameter节圆锥 pitch cone节圆锥角 pitch cone angle解析设计 analytical design紧边 tight-side紧固件 fastener径节 diametral pitch径向 radial direction径向当量动载荷 dynamic equivalent radial load径向当量静载荷 static equivalent radial load径向基本额定动载荷 basic dynamic radial load rating 径向基本额定静载荷 basic static radial load tating径向接触轴承 radial contact bearing径向平面 radial plane径向游隙 radial internal clearance径向载荷 radial load径向载荷系数 radial load factor径向间隙 clearance静力 static force静平衡 static balance静载荷 static load静密封 static seal如有你有帮助，请购买下载，谢谢！局部自由度 passive degree of freedom矩阵 matrix矩形螺纹 square threaded form锯齿形螺纹 buttress thread form矩形牙嵌式离合器 square-jaw positive-contact clutch绝对尺寸系数 absolute dimensional factor绝对运动 absolute motion绝对速度 absolute velocity均衡装置 load balancing mechanism抗压强度 compression strength开口传动 open-belt drive开式链 open kinematic chain开链机构 open chain mechanism可靠度 degree of reliability可靠性 reliability可靠性设计 reliability design, RD空气弹簧 air spring空间机构 spatial mechanism空间连杆机构 spatial linkage空间凸轮机构 spatial cam空间运动副 spatial kinematic pair空间运动链 spatial kinematic chain空转 idle宽度系列 width series框图 block diagram雷诺方程Reynolds‘s equation离心力 centrifugal force离心应力 centrifugal stress离合器 clutch离心密封 centrifugal seal理论廓线 pitch curve理论啮合线 theoretical line of action隶属度 membership力 force力多边形 force polygon力封闭型凸轮机构 force-drive (or force-closed) cam mechanism 力矩 moment力平衡 equilibrium力偶 couple力偶矩 moment of couple连杆 connecting rod, coupler连杆机构 linkage连杆曲线 coupler-curve连心线 line of centers链 chain链传动装置 chain gearing 链轮 sprocket ; sprocket-wheel ; sprocket gear ; chain wheel 联组V 带 tight-up V belt联轴器 coupling ; shaft coupling两维凸轮 two-dimensional cam临界转速 critical speed六杆机构 six-bar linkage龙门刨床 double Haas planer轮坯 blank轮系 gear train螺杆 screw螺距 thread pitch螺母 screw nut螺旋锥齿轮 helical bevel gear螺钉 screws螺栓 bolts螺纹导程 lead螺纹效率 screw efficiency螺旋传动 power screw螺旋密封 spiral seal螺纹 thread (of a screw)螺旋副 helical pair螺旋机构 screw mechanism螺旋角 helix angle螺旋线 helix ,helical line绿色设计 green design ; design for environment马耳他机构 Geneva wheel ; Geneva gear马耳他十字 Maltese cross脉动无级变速 pulsating stepless speed changes脉动循环应力 fluctuating circulating stress脉动载荷 fluctuating load铆钉 rivet迷宫密封 labyrinth seal密封 seal密封带 seal belt密封胶 seal gum密封元件 potted component密封装置 sealing arrangement面对面安装 face-to-face arrangement面向产品生命周期设计 design for product`s life cycle, DPLC 名义应力、公称应力 nominal stress模块化设计 modular design, MD模块式传动系统 modular system模幅箱 morphology box模糊集 fuzzy set模糊评价 fuzzy evaluation模数 module如有你有帮助，请购买下载，谢谢！摩擦 friction摩擦角 friction angle摩擦力 friction force摩擦学设计 tribology design, TD摩擦阻力 frictional resistance摩擦力矩 friction moment摩擦系数 coefficient of friction摩擦圆 friction circle磨损 abrasion ;wear; scratching末端执行器 end-effector目标函数 objective function耐腐蚀性 corrosion resistance耐磨性 wear resistance挠性机构 mechanism with flexible elements 挠性转子 flexible rotor内齿轮 internal gear内齿圈 ring gear内力 internal force内圈 inner ring能量 energy能量指示图 viscosity逆时针 counterclockwise (or anticlockwise) 啮出 engaging-out啮合 engagement, mesh, gearing啮合点 contact points啮合角 working pressure angle啮合线 line of action啮合线长度 length of line of action啮入 engaging-in牛头刨床 shaper凝固点 freezing point; solidifying point扭转应力 torsion stress扭矩 moment of torque扭簧 helical torsion spring诺模图 NomogramO 形密封圈密封 O ring seal盘形凸轮 disk cam盘形转子 disk-like rotor抛物线运动 parabolic motion疲劳极限 fatigue limit疲劳强度 fatigue strength偏置式 offset偏( 心) 距 offset distance偏心率 eccentricity ratio偏心质量 eccentric mass偏距圆 offset circle 偏心盘 eccentric偏置滚子从动件 offset roller follower偏置尖底从动件 offset knife-edge follower偏置曲柄滑块机构 offset slider-crank mechanism拼接 matching评价与决策 evaluation and decision频率 frequency平带 flat belt平带传动 flat belt driving平底从动件 flat-face follower平底宽度 face width平分线 bisector平均应力 average stress平均中径 mean screw diameter平均速度 average velocity平衡 balance平衡机 balancing machine平衡品质 balancing quality平衡平面 correcting plane平衡质量 balancing mass平衡重 counterweight平衡转速 balancing speed平面副 planar pair, flat pair平面机构 planar mechanism平面运动副 planar kinematic pair平面连杆机构 planar linkage平面凸轮 planar cam平面凸轮机构 planar cam mechanism平面轴斜齿轮 parallel helical gears普通平键 parallel key其他常用机构 other mechanism in common use起动阶段 starting period启动力矩 starting torque气动机构 pneumatic mechanism奇异位置 singular position起始啮合点 initial contact , beginning of contact气体轴承 gas bearing千斤顶 jack嵌入键 sunk key强迫振动 forced vibration切齿深度 depth of cut曲柄 crank曲柄存在条件 Grashoff`s law曲柄导杆机构 crank shaper (guide-bar) mechanism曲柄滑块机构 slider-crank (or crank-slider) mechanism 曲柄摇杆机构 crank-rocker mechanism如有你有帮助，请购买下载，谢谢！曲齿锥齿轮 spiral bevel gear曲率 curvature曲率半径 radius of curvature曲面从动件 curved-shoe follower曲线拼接 curve matching曲线运动 curvilinear motion曲轴 crank shaft驱动力 driving force驱动力矩 driving moment (torque)全齿高 whole depth权重集 weight sets球 ball球面滚子 convex roller球轴承 ball bearing球面副 spheric pair球面渐开线 spherical involute球面运动 spherical motion球销副 sphere-pin pair球坐标操作器 polar coordinate manipulator燃点 spontaneous ignition热平衡 heat balance; thermal equilibrium人字齿轮 herringbone gear冗余自由度 redundant degree of freedom柔轮 flexspline柔性冲击 flexible impulse; soft shock柔性制造系统 flexible manufacturing system; FMS柔性自动化 flexible automation润滑油膜 lubricant film润滑装置 lubrication device润滑 lubrication润滑剂 lubricant三角形花键 serration spline三角形螺纹 V thread screw三维凸轮 three-dimensional cam三心定理 Kennedy`s theorem砂轮越程槽 grinding wheel groove砂漏 hour-glass少齿差行星传动 planetary drive with small teeth difference 设计方法学 design methodology设计变量 design variable设计约束 design constraints深沟球轴承 deep groove ball bearing生产阻力 productive resistance升程 rise升距 lift实际廓线 cam profile 十字滑块联轴器double slider coupling; Oldham‘s coupling 矢量 vector输出功 output work输出构件 output link输出机构 output mechanism输出力矩 output torque输出轴 output shaft输入构件 input link数学模型 mathematic model实际啮合线 actual line of action双滑块机构 double-slider mechanism, ellipsograph双曲柄机构 double crank mechanism双曲面齿轮 hyperboloid gear双头螺柱 studs双万向联轴节 constant-velocity (or double) universal joint 双摇杆机构 double rocker mechanism双转块机构 Oldham coupling双列轴承 double row bearing双向推力轴承 double-direction thrust bearing松边 slack-side顺时针 clockwise瞬心 instantaneous center死点 dead point四杆机构 four-bar linkage速度 velocity速度不均匀( 波动) 系数 coefficient of speed fluctuation 速度波动 speed fluctuation速度曲线 velocity diagram速度瞬心 instantaneous center of velocity塔轮 step pulley踏板 pedal台钳、虎钳 vice太阳轮 sun gear弹性滑动 elasticity sliding motion弹性联轴器 elastic coupling ; flexible coupling弹性套柱销联轴器 rubber-cushioned sleeve bearing coupling 套筒 sleeve梯形螺纹 acme thread form特殊运动链 special kinematic chain特性 characteristics替代机构 equivalent mechanism调节 modulation, regulation调心滚子轴承 self-aligning roller bearing调心球轴承 self-aligning ball bearing调心轴承 self-aligning bearing调速 speed governing如有你有帮助，请购买下载，谢谢！调速电动机 adjustable speed motors调速系统 speed control system调压调速 variable voltage control调速器 regulator, governor铁磁流体密封 ferrofluid seal停车阶段 stopping phase停歇 dwell同步带 synchronous belt同步带传动 synchronous belt drive凸的，凸面体 convex凸轮 cam凸轮倒置机构 inverse cam mechanism凸轮机构 cam , cam mechanism凸轮廓线 cam profile凸轮廓线绘制 layout of cam profile凸轮理论廓线 pitch curve凸缘联轴器 flange coupling图册、图谱 atlas图解法 graphical method推程 rise推力球轴承 thrust ball bearing推力轴承 thrust bearing退刀槽 tool withdrawal groove退火 anneal陀螺仪 gyroscopeV 带 V belt外力 external force外圈 outer ring外形尺寸 boundary dimension万向联轴器 Hooks coupling ; universal coupling 外齿轮 external gear弯曲应力 beading stress弯矩 bending moment腕部 wrist往复移动 reciprocating motion往复式密封 reciprocating seal网上设计 on-net design, OND微动螺旋机构 differential screw mechanism位移 displacement位移曲线 displacement diagram位姿 pose , position and orientation稳定运转阶段 steady motion period稳健设计 robust design蜗杆 worm蜗杆传动机构 worm gearing蜗杆头数 number of threads 蜗杆直径系数 diametral quotient蜗杆蜗轮机构 worm and worm gear蜗杆形凸轮步进机构 worm cam interval mechanism蜗杆旋向 hands of worm蜗轮 worm gear涡圈形盘簧 power spring无级变速装置 stepless speed changes devices无穷大 infinite系杆 crank arm, planet carrier现场平衡 field balancing向心轴承 radial bearing向心力 centrifugal force相对速度 relative velocity相对运动 relative motion相对间隙 relative gap象限 quadrant橡皮泥 plasticine细牙螺纹 fine threads销 pin消耗 consumption小齿轮 pinion小径 minor diameter橡胶弹簧 balata spring修正梯形加速度运动规律 modified trapezoidal acceleration motion 修正正弦加速度运动规律 modified sine acceleration motion斜齿圆柱齿轮 helical gear斜键、钩头楔键 taper key泄漏 leakage谐波齿轮 harmonic gear谐波传动 harmonic driving谐波发生器 harmonic generator斜齿轮的当量直齿轮 equivalent spur gear of the helical gear心轴 spindle行程速度变化系数 coefficient of travel speed variation行程速比系数 advance-to return-time ratio行星齿轮装置 planetary transmission行星轮 planet gear行星轮变速装置 planetary speed changing devices行星轮系 planetary gear train形封闭凸轮机构 positive-drive (or form-closed) cam mechanism虚拟现实 virtual reality虚拟现实技术 virtual reality technology, VRT虚拟现实设计 virtual reality design, VRD虚约束 redundant (or passive) constraint许用不平衡量 allowable amount of unbalance许用压力角 allowable pressure angle如有你有帮助，请购买下载，谢谢！许用应力 allowable stress; permissible stress悬臂结构 cantilever structure悬臂梁 cantilever beam循环功率流 circulating power load旋转力矩 running torque旋转式密封 rotating seal旋转运动 rotary motion选型 type selection压力 pressure压力中心 center of pressure压缩机 compressor压应力 compressive stress压力角 pressure angle牙嵌式联轴器 jaw (teeth) positive-contact coupling雅可比矩阵 Jacobi matrix摇杆 rocker液力传动 hydrodynamic drive液力耦合器 hydraulic couplers液体弹簧 liquid spring液压无级变速 hydraulic stepless speed changes液压机构 hydraulic mechanism一般化运动链 generalized kinematic chain移动从动件 reciprocating follower移动副 prismatic pair, sliding pair移动关节 prismatic joint移动凸轮 wedge cam盈亏功 increment or decrement work应力幅 stress amplitude应力集中 stress concentration应力集中系数 factor of stress concentration应力图 stress diagram应力—应变图 stress-strain diagram优化设计 optimal design油杯 oil bottle油壶 oil can油沟密封 oily ditch seal有害阻力 useless resistance有益阻力 useful resistance有效拉力 effective tension有效圆周力 effective circle force有害阻力 detrimental resistance余弦加速度运动 cosine acceleration (or simple harmonic) motion 预紧力 preload原动机 primer mover圆带 round belt圆带传动 round belt drive 圆弧齿厚 circular thickness圆弧圆柱蜗杆 hollow flank worm圆角半径 fillet radius圆盘摩擦离合器 disc friction clutch圆盘制动器 disc brake原动机 prime mover原始机构 original mechanism圆形齿轮 circular gear圆柱滚子 cylindrical roller圆柱滚子轴承 cylindrical roller bearing圆柱副 cylindric pair圆柱式凸轮步进运动机构 barrel (cylindric) cam圆柱螺旋拉伸弹簧 cylindroid helical-coil extension spring圆柱螺旋扭转弹簧 cylindroid helical-coil torsion spring圆柱螺旋压缩弹簧 cylindroid helical-coil compression spring 圆柱凸轮 cylindrical cam圆柱蜗杆 cylindrical worm圆柱坐标操作器 cylindrical coordinate manipulator圆锥螺旋扭转弹簧 conoid helical-coil compression spring圆锥滚子 tapered roller圆锥滚子轴承 tapered roller bearing圆锥齿轮机构 bevel gears圆锥角 cone angle原动件 driving link约束 constraint约束条件 constraint condition约束反力 constraining force跃度 jerk跃度曲线 jerk diagram运动倒置 kinematic inversion运动方案设计 kinematic precept design运动分析 kinematic analysis运动副 kinematic pair运动构件 moving link运动简图 kinematic sketch运动链 kinematic chain运动失真 undercutting运动设计 kinematic design运动周期 cycle of motion运动综合 kinematic synthesis运转不均匀系数 coefficient of velocity fluctuation运动粘度 kenematic viscosity载荷 load载荷—变形曲线 load—deformation curve载荷—变形图 load—deformation diagram窄V 带 narrow V belt如有你有帮助，请购买下载，谢谢！毡圈密封 felt ring seal展成法 generating张紧力 tension张紧轮 tension pulley振动 vibration振动力矩 shaking couple振动频率 frequency of vibration振幅 amplitude of vibration正切机构 tangent mechanism正向运动学 direct (forward) kinematics正弦机构 sine generator, scotch yoke织布机 loom正应力、法向应力 normal stress制动器 brake直齿圆柱齿轮 spur gear直齿锥齿轮 straight bevel gear直角三角形 right triangle直角坐标操作器 Cartesian coordinate manipulator 直径系数 diametral quotient直径系列 diameter series直廓环面蜗杆 hindley worm直线运动 linear motion直轴 straight shaft质量 mass质心 center of mass执行构件 executive link; working link质径积 mass-radius product智能化设计 intelligent design, ID中间平面 mid-plane中心距 center distance中心距变动 center distance change中心轮 central gear中径 mean diameter终止啮合点 final contact, end of contact周节 pitch周期性速度波动 periodic speed fluctuation周转轮系 epicyclic gear train肘形机构 toggle mechanism轴 shaft轴承盖 bearing cup轴承合金 bearing alloy轴承座 bearing block轴承高度 bearing height轴承宽度 bearing width轴承内径 bearing bore diameter轴承寿命 bearing life 轴承套圈 bearing ring轴承外径 bearing outside diameter轴颈 journal轴瓦、轴承衬 bearing bush轴端挡圈 shaft end ring轴环 shaft collar轴肩 shaft shoulder轴角 shaft angle轴向 axial direction轴向齿廓 axial tooth profile轴向当量动载荷 dynamic equivalent axial load轴向当量静载荷 static equivalent axial load轴向基本额定动载荷 basic dynamic axial load rating轴向基本额定静载荷 basic static axial load rating轴向接触轴承 axial contact bearing轴向平面 axial plane轴向游隙 axial internal clearance轴向载荷 axial load轴向载荷系数 axial load factor轴向分力 axial thrust load主动件 driving link主动齿轮 driving gear主动带轮 driving pulley转动导杆机构 whitworth mechanism转动副 revolute (turning) pair转速 swiveling speed ; rotating speed转动关节 revolute joint转轴 revolving shaft转子 rotor转子平衡 balance of rotor装配条件 assembly condition锥齿轮 bevel gear锥顶 common apex of cone锥距 cone distance锥轮 bevel pulley; bevel wheel锥齿轮的当量直齿轮 equivalent spur gear of the bevel gear 锥面包络圆柱蜗杆 milled helicoids worm准双曲面齿轮 hypoid gear子程序 subroutine子机构 sub-mechanism自动化 automation自锁 self-locking自锁条件 condition of self-locking自由度 degree of freedom, mobility总重合度 total contact ratio总反力 resultant force如有你有帮助，请购买下载，谢谢！总效率 combined efficiency; overall efficiency组成原理 theory of constitution组合齿形 composite tooth form组合安装 stack mounting组合机构 combined mechanism阻抗力 resistance最大盈亏功 maximum difference work between plus and minus work纵向重合度 overlap contact ratio纵坐标 ordinate组合机构 combined mechanism最少齿数 minimum teeth number最小向径 minimum radius作用力 applied force坐标系 coordinate frame。

人体行走过程中上肢运动仿真及生物力学特征分析

第43卷第8期 2009年8月上海交通大学学报JOU RN AL O F SH AN G HA I JIA OT O N G U N IV ERSIT YVol.43No.8 Aug.2009收稿日期:2008 09 02基金项目:国家自然科学基金重点项目资助(30530230,30470455);上海市体育局科研攻关与科技服务项目资助(07J T018)作者简介:王洪生(1986 ),男,江西上饶人,硕士生.主要研究方向为人体生物力学、人体运动学.王成焘(联系人),男,教授,博士生导师,电话(T el.):021 ********;E m ail:trib@s .文章编号:1006 2467(2009)08 1302 05人体行走过程中上肢运动仿真及生物力学特征分析王洪生, 白雪岭, 张希安, 张琳琳, 王成焘(上海交通大学机械与动力工程学院,上海200240)摘要:为了分析人体行走过程中上肢运动状态的影响因素,对正常步态下人体上肢运动仿真模型与理想单摆模型进行了对比分析.以7名(4男,3女)步态无异常的志愿者为研究对象,采集人体测量学参数,建立上肢理想单摆模型.采用运动捕捉系统及肌电测量系统,对志愿者常速(1.2m/s)步行下的上肢运动以及肩关节周围6组肌肉的肌电信号进行同步测量,并基于所测运动学参数对人体行走中上肢运动进行仿真,计算实际肩关节角位移和角速度,分析相关肌肉的肌电信号特征.理想模型与实际测量结果比较表明,不同志愿者在同速行走过程中上肢的摆动周期相近,各相关肌肉肌电信号随摆臂而周期性变化,上肢实际最大摆动角速度均大于理想单摆角速度.证明摆臂过程中肩关节周围相关肌群驱动力大于肩关节阻尼.关键词:上肢;肌电信号;步态;运动捕捉中图分类号:R 318.01 文献标识码:AKinematics Simulation and Biomechanics Characteristic Analysis of U pper Extremity during Human WalkingWA N G H ong sheng , BA I X ue ling , ZH A N G X i an, ZH AN G L in lin, WAN G Cheng tao (School of M echanical Eng ineer ing ,Shanghai Jiaotong U niv er sity,Shanghai 200240,China)Abstract:In order to investig ate the factors that influence the mo vem ent condition o f upper extremity dur ing hum an walking,a co mparative study betw een the ex perimental conditio n and the ideal pendulum mo del condition w as co nducted.Firstly,sev en health participants (4males and 3females)w ere inv olved in the ex periment and the pendulum m odels w ere built according to their anthro pom etric parameters.Secondly,the markers coordinatio n and the EM G of related m uscles w ere simultaneously m easured w hen the partici pant w as w alking on a tr eadm ill w ith a daily v elo city of 1.2m/s.Based on the captured signals,the angle and angular velocity of the sho ulder joint w as calculated and the actual angular velocity w as co mpared w ith the v elo city of the upper extremity pendulum mo del.Also an analy sis w as undertaken for the recorded EM G.The study sho ws that the actual maxim um angular velocity of shoulder joint ex ceeds those of the virtual pendulum s and the EM G of the related m uscles w as cycling according to the periods of sw ing.The ex periment also indicates that the actual periods o f upper limb swing represent a level of similarity amo ng different participants;the related muscular forces contribute larg er motivity than the damp of the shoulder joint during human w alking in a no rmal speed.Key words:upper ex trem ity ;electro myo graphy (EM G)sig nals;g ait;mo tion capture正常人体步行过程中,上肢伴随下肢的运动而做周期摆动.Wagenaar等[1]认为,人体在低速运动过程中上肢的习惯摆动周期与上肢的固有频率(质量与质心到肩关节的长度的函数)有关;而在高速过程中上肢摆动频率主要与下肢的频率有关.Webb 等[2]在对人体上肢运动特性的研究中引入了虚拟单摆理论,分析了步频与人体上肢单摆频率的关系,并认为单摆假设将在上肢运动研究中扮演重要角色. Bertram等[3]对人体上肢单摆模型进行了进一步研究,并从能量角度对模型仿真性能加以探讨.而基于上肢单摆模型的研究表明[4],人体肌肉能量消耗主要用来改变人体的质心位置.Neptune等[5]提出肌肉的能量输出不仅用于双脚支撑阶段时人体质心位置的改变,还用于单脚支撑阶段质心位置抬高. Gutnik等[6]把步态过程中人体上肢运动与虚拟单摆运动进行了比较,认为肌肉对上肢运动具有一定的影响.然而,以上研究没有在人体步态测量过程中同步进行主要肌肉群的肌电信号测量.本文在步态试验中对肌电信号进行同步测量,与理想单摆模型进行对比,分析上肢肌肉群对人体行走过程中上肢摆臂运动的影响.1 试验方法1.1 试验对象在上海交通大学机械与动力工程学院师生中挑选7名身体健康并步态无异常的志愿者(4男3女)作为研究对象(试验之前他们均不知道本试验的直接目的).测量志愿者的人体测量学参数(见表1),包括:身高、体重、前臂长度、上臂长度以及手长;前臂长度为桡骨茎突到外上髁距离、上臂为肩峰到外上髁,手为桡骨茎突到中指指尖距离,臂长取为肩峰到桡骨茎突.表1 志愿者人体测量学基本参数Tab.1 Anthropom etric parameters of participants试验对象(编号)身高/m体重/kg上臂长/m前臂长/m手长/m#1 1.76363.20.3150.2450.195男性#2 1.63350.20.3000.2180.177#3 1.73563.50.3120.2550.190#4 1.81780.10.3250.2620.200#5 1.65455.20.2820.2250.178女性#6 1.58746.50.2850.2100.175 #7 1.72153.40.3170.2450.1801.2 试验设备及方案本试验采用Optotrak Certus运动捕捉系统对人体行走过程中上肢运动进行运动捕捉.如图1所示,2个Marker刚体(每个刚体至少由3个Marker 点组成)通过自黏性纱布分别固结在前臂、上臂的外侧,另一个刚体贴附于胸骨,以参考计算上臂摆动角度.由于手腕关节在行走过程中运动幅度微小,故可把手与前臂视为同一个刚体.对于不易采集的各关键解剖特性点,采用Optotrak Certus运动捕捉系统的虚拟工具进行虚拟M arker设置(虚拟M arker所对应刚体的位置不变),分别对上肢运动相关的6个解剖特征点(肩峰、外上髁、内上髁、桡骨茎突、尺骨茎突、中节指关节)设置虚拟Marker点,并测量贴附于试验对象上肢主动发光Marker点的三维运动轨迹.上肢的运动的捕捉频率为30H z,测量误差在0.1m m以内.图1 前臂/上臂刚体及创建的虚拟M arker(白色的亮点示意)F ig.1 T he attached M arker cluster s of for earm/upper arm&the anat omical land mar ker s(w hite highlig hts)采用8 通道肌电测试系统对摆臂过程中肩关节周围6块主要肌肉(三角肌前部、胸大肌锁骨部、肱二头肌、背阔肌、大圆肌、三角肌后部)的肌电信号进行同步测量,试验过程详见文献[7].1.3 试验过程首先对试验对象进行人体学参数测量,然后按以上制定的M ar ker点和肌电电极方案依次对其进行贴附.志愿者上身穿短T恤或背心.在捕捉试验开始之前调节跑步机速度至1.2m/s,每位试验者都将进行至少4次适应性练习,以适应试验室的光线、温度、跑步机及其他仪器设备.要求试验者以自然放松的状态走,然后开始捕捉测量;每位试验者同样动作循环20次.2 结果及数据分析2.1 上肢单摆模型的运动分析上肢运动过程中单摆的质量为上肢的总质量,1303第8期王洪生,等:人体行走过程中上肢运动仿真及生物力学特征分析单摆长度为上肢质心到肩关节距离.如图2所示,前屈/后伸过程中质心落在上肢体以外,具体位置确定如下[8]:x = (m ix i )m iy = (mi y i )mil =(x 2+y 2)(1)式中:m i 为各部位的质量;x i 为m i 质心到支点(肩关节)的水平距离;y i 为m i 质心到支点(肩关节)的垂直距离;l 为单摆的长度.得到最大前屈位置或最大后伸位置的等效单摆的质量与长度,则在最低点的转速:I max =m l 2maxE potential =mg (L -l max )max =2E potential /I max(2)式中:l max 为平衡位置单摆最大臂长;L 为平衡位置单摆臂长;E potential 为上臂最高点(最大前屈)势能,g 为重力加速度;I max 为平衡位置转动惯量; max 为单摆最大角速度.根据人体惯性参数的国际标准[9],把已测得的人体参数代入如下的二元回归方程:y =B 0+B 1X 1+B 2X 2(3)图2 上肢摆动前屈/后伸过程F ig.2 T he fo rw ard flex io n/backwar d ex tensiono f upper limb式中:X 1、X 2分别为试验对象的体重与身高;B 0、B 1、B 2为方程系数(与性别有关).由式(3)计算出试验者上肢各部位的质量、质心位置.将上肢各部位的质量、质心位置代入式(1)可得各位试验者上肢的l max 、L 和I max (见表2).最大前屈/后伸垂直位置可由三维捕捉系统(NDI)测量得到;理想单摆模型的最大前屈势能全部转化为摆动最低点的动能,把所得数据分别代入式(2)可得单摆平均最大角速度 max ,结果如表2所示.表中质心测量起点为:上臂,即桡骨点;前臂,即桡骨茎突点;手,即中指指尖点.表2 志愿者上肢基本惯性参数及计算所得单摆模型参数Tab.2 The inertial parameters of upper limb and the calculated parameters of virtual pendulum试验对象(编号)质心位置(m)/质量(k g)上臂前臂手l ma x /m I ma x /(k g !m 2) max /(rad !s -1)#10.167/1.750.137/0.910.116/0.470.2810.2477 1.024男性#20.154/1.350.124/0.690.110/0.380.2470.1995 1.457#30.164/1.760.135/0.910.116/0.460.2750.2454 1.214#40.174/2.260.147/1.200.122/0.540.3020.2832 1.078#50.158/1.450.122/0.650.116/0.270.2540.2024 1.201女性#60.150/1.190.116/0.520.112/0.250.2420.1878 1.054#70.161/1.310.123/0.630.116/0.280.2650.21841.1652.2 实际运动测量数据分析利用NDI 将测得的各解剖特性点的运动学参数以及肌电测量仪(Bo rtec)同步采集的目标肌肉的肌电信号保存为*.c3d 格式的文件.然后,将该文件导入Visual3D 中建立对象的三维骨架模型,并进行运动计算,计算正常行走过程中上肢摆动的角位移、角速度.其中,典型运动阶段如图3所示.图4所示为正常行走过程中上肢摆动状态下的肩关节角位移和角速度曲线.其中,#1为所测4名男性试验者运动学参数平均值,#2为3名女性试图3 V isual3D 上肢模型摆动的典型阶段F ig.3 T he mo del of ty pical perio ds dur ing upperlimb swing1304上海交通大学学报第43卷(a)#1试验者步行时手部解剖特性点垂直位置变化(b)#2试验者步行时手部解剖特性点垂直位置变化(c)#1试验者步行时肩关节矢状面角度(d)#2试验者步行时肩关节矢状面角度(e)#1试验者步行时肩关节矢状面角速度(f)#2试验者步行时肩关节矢状面角速度图4 试验对象的中节指关节点垂直位移、肩关节角位移与角速度曲线F ig.4 T he vert ical displacement o f PM,ang ular displacement and velocity of shoulder joint fo r participants验者的平均值,采样频率为30H z.可见:正常速度(1.2m/s)下不同对象同速步行时,摆臂周期具有一定统一性[(1.1∀0.08)s],但摆臂幅度以及角速度有较大差异.#1步行过程中肩关节最大转角为(0.17∀0.01)rad与(-0.12∀0.01)rad(后伸);最大角速度为(0.64∀0.11)rad/s与(-0.88∀0.07)rad/s(后伸).#2肩关节最大转角为(0.20∀0.02)rad与(-0.13∀0.01)rad(后伸);最大角速度为(0.84∀0.12)rad/s与(1.00∀0.05)rad/s(后伸).将实测结果与理想单摆模型计算结果进行比较发现,人体上肢理想单摆模型计算得到的矢状面上肢运动学参数与实际结果偏差较大.2.3 肌电信号分析本文对志愿者正常行走过程中上肢主要肌肉的肌电信号进行了同步采集,采集频率为900H z.结果表明,在步态过程中,肌电信号呈现出周期性变化特征.图5所示为一个步态周期下,志愿者正常行走过程中肩关节主要肌肉的肌电信号,其中,高频信号已经过整流与低通滤波处理.由图可见,1个周期内各肌电信号的变化趋势:前屈阶段(0~0.75s)三角肌前头、三角肌后头受到较大刺激,肌电信号分别从0.02mV增至(0.103∀0.007)mV(三角肌前头)、(0.081∀0.006)mV(三角肌后头);大圆肌有平均1305 第8期王洪生,等:人体行走过程中上肢运动仿真及生物力学特征分析0.37mV 的肌电信号;后伸阶段(0.75~1.17s)大圆肌肌电信号从0.037m V 增大到0.105mV,三角肌前/后头也分别受到0.03mV 水平的肌电刺激;在整个周期中,背阔肌、肱二头肌及胸大肌的肌电信号较小.图5 上肢摆动中肩关节主要肌肉的肌电信号Fig.5 EM G o f the main muscles related to upperlimb s movement3 讨论男性试验者上肢单摆模型的平均最大角速度为0.51rad/s,而实际所测最大前屈角速度为(0.64∀0.11)r ad/s;女性试验者上肢单摆模型最大角速度为0.73rad/s,而实际所测最大前屈角速度为(0.84∀0.12)r ad/s.比较上述数据,即人体实际最大摆臂速度大于无阻尼下的单摆速度.所测肌电信号反映了上肢肌肉对摆臂的作用情况,即前屈阶段三角肌前/后头发出较大的肌肉力、而后伸阶段大圆肌对上肢施加了较大的驱动力.肱二头肌肌电信号较小,说明在摆臂过程中肘关节屈伸幅度较小;胸大肌肌电信号较小,说明摆臂过程中上肢外展、外旋(即冠状面、横截面运动)幅度较小.背阔肌对上臂的作用主要为使臂内收、内旋和后伸,其信号微弱,说明摆臂过程中内收、内旋的阻力较小.同时,由于前屈时较大的势能转化为后伸的动能,使得在正常行走中需要背阔肌作为后伸原动力较小.上肢摆动状态受重力、相关肌肉力和肩关节关节阻尼的综合影响,由实际与理想模型的比较结果可知,肌肉力驱动作用大于关节阻尼,则实际速度大于理想模型速度.4 结语本文基于运动捕捉系统和肌电测量系统,以7名无步态异常的高校师生为试验对象,对其进行了正常步态过程中上肢运动的运动学测量,并同步测量了相关肌肉的肌电信号,避免了运动与肌电分开测量时所带来的误差.将实际运动与上肢理想单摆模型运动进行比较,得出正常步态(1.2m/s)三角肌、大圆肌对肩关节驱动力作用大于关节阻尼,且实际摆臂角速度大于无阻尼状态的理想单摆模型角速度.文中建立的一整套试验方案对以后人体上肢运动测量试验有一定的指导作用,对人体上肢运动状态的深入研究、人体运动机制研究、脑偏瘫诊断、康复治疗等领域具有深远意义.参考文献:[1] Wag enaar R C,v an Emmer ik R E.R eso nant fr equencies of arms and legs identify different w alking patterns [J].Journal of Biomechanical ,2000,33(7):853 861.[2] Webb D,T uttle R H,Baksh M .Pendular activ ity o fhuman upper limbs during slo w and no rmal w alking [J].American Journal of Physical Anthropology ,1994,93(4):477 489.[3] Bert ram J E,Chang Y H.M echanical energ y oscillations o f tw o brachiatio n g aits:M easurement and simu latio n [J].Am erican Journal of Physical Anthropology ,2001,115(4):319 326.[4] Kuo A D.Ener getics o f actively pow ered lo co motionusing the simplest w alking mo del [J].Journal of Bio mechanical Engineering ,2002,124(2):113 120.[5] Neptune R R,Zajac F E,Kautz S A.M uscle mechanical w or k r equir ements during no rmal w alking :T he en er getic cost of r aising the bo dy s center of mass is sig nificant [J].Journal of Biomechanics ,2004,37(6):817 825.[6] Gutnik B,M ackie H ,H udson G,et al .H ow clo se toa pendulum is human upper limb mov ement during walking ?[J].Journal of Comparative Human Biology ,2005,56(1):35 49.[7] Luttg ens K ,Hamilto n N.Kinesio lo gy :Scientif ic basisof human motio n [M ].Bosto n:W CB M cGr aw H ill,1997.[8] T imo shenko S,Y oung D H.Eng ineer ing mechanics[M ].4th edition .N ew Y or k:M cGr aw H ill,1956.[9] 刘静民.GB/T 17245 2004成年人人体惯性参数[M ].北京:中国标准出版社,2004.1306上海交通大学学报第43卷。

杆组法作运动分析的思路.

RRP
RPR
Kinematic analysis by analytical
methods
Given :
the length of AB is l AB , the position of A(xA,y A ), the velocity and acceleration of point A is vA, aA, also knownφ，ω，ε。
Kinematic analysis by analytical methods
杆组法 ---- 杆组法作运动分析的思路
机构的运动部分是由原动件 --- 单杆构件和基本杆组组成的。因此只要分别对单杆构件和各基本杆组用解析法进行运动分析并编制成相应的子程序，然后按照实际机构的结构组成情况，依次调用所需的子程序，就可完成对整个机构的运动分析。
2.Kinematic analysis by analytical methods
Example
已知:图示六杆机构中，已知各杆长度LAB=80mm， LBC=260mm, LCD=300mm, LDE=400mm , LEF=460mm, 曲柄AB逆时针方向等角速度转动，ω1=40rad/s; 求：该机构在一个运动循环中，滑块5的位移SF ，速度 vF ，加速度aF ,及构件2，3， 4的角速度ω2,ω3,ω4 ，角加速度ε2,ε3,ε4 。
Kinematic analysis by analytical methods
Types of grade II Assur group 工程实际中所用的机构多为II 级机构，它们所含的基本杆组是II级杆组，即双杆组。双杆组最常见的有三种型式： RRR双杆组 RRP双杆组 RPR双杆组

kinematics词根

"Kinematics" 一词源于希腊语，其词根为 "kinesis"。

在希腊语中，"kinesis" 意为 "运动" 或 "移动"。

因此，"kinematics" 表示研究物体运动的学科，着重于描述物体的位置、速度、加速度等运动相关的性质，而不考虑导致运动的力的原因（这部分内容由动力学来研究）。

"Kinematics" 通常用于物理学领域，特别是在描述刚体、粒子或系统的运动时。

研究物体的位置、速度和加速度等参数可以提供关于物体如何运动的重要信息，而这些信息对于工程、天文学、生物学等领域都有广泛的应用。

希腊语的 "kinesis" 词根还出现在其他一些与运动有关的术语中，例如：
1.Cinema（电影）：来自希腊语 "kinema"，意为 "运动的图像"。

2.Kinesiology（运动学）：表示研究人体运动的学科，与生理学和解剖学相
结合，以理解人体运动的机制和原理。

3.Telekinesis（心灵动能）：表示通过思维或意念而非物理力量来影响物体的
能力。

机械原理重要名词术语中英文对照表

机械原理重要名词术语中英文对照表Aabsolute motion 绝对运动absolute velocity 绝对速度acceleration加速度acceleration analysis加速度分析acceleration diagram 加速度曲线addendum 齿顶高addendum circle 齿顶圆additional mechanism附加机构allowable amount of unbalance 许用不平衡量allowable pressure angle 许用压力角amount of unbalance 不平衡量amplitude of vibration 振幅analytical design 解析设计analysis of mechanism机构分析angle of contact 包角angle of engagement 啮合角angular acceleration 角加速度angular velocity 角速度angular velocity ratio 角速比aperiodic speed fluctuation 非周期性速度波动applied force 作用力arm 臂部archimedes worm 阿基米德蜗杆assembly condition 装配条件automation 自动化axial thrust load 轴向分力Bback angle 背锥角back cone 背锥back cone distance 背锥距backlash 侧系balance mass, quality of mass 平衡质量balance of balance机构平衡balance of machinery 机械平衡balance of shaking force 惯性力平衡balance 平衡balancing machine 平衡机balancing quality 平衡品质balancing speed 平衡转速balance of rotor 转子平衡base circle基圆base cone 基圆锥base cylinder 基圆柱base pitch 基圆齿距belt pulley 带轮belt pulley 皮带轮belt drives 带传动bevel gears 圆锥齿轮机构bevel gear 锥齿轮blank 齿轮轮坯block diagram 框图body guidance mechanism 刚体导引机构Ccam with oscillating follower 摆动从动件运动规律cam profile 实际廓线cam 凸轮cams, cam mechanism 凸轮机构cam profile 凸轮(实际)廓线cartesian coordinate manipulator 直角坐标操作器centrifugal force 离心力center distance 中心距center distance change 中心距变动central gear 中心轮chain wheel 链轮characteristics 特性circular pitch 齿距clearance 顶隙clearance 径向间歇closed kinematic chain 闭式运动链closed chain mechanism 闭式链机构coefficient of speed fluctuation机械运转不均匀系数coefficient of friction 摩擦系数coefficient of speed fluctuation 速度波动系数coefficient of travel speed variation, advance-to return-time ratio 行程速比系数coefficient of velocity fluctuation 运动不均匀系数coincident points 重合点bine in parallel 并联式组合mon normal line 公法线pound hinge 复合铰链pound bining复合式组合pound screw mechanism复式螺旋机构pound gear train 复合轮系plex mechanism复杂机构puter aided design计算机辅助设计puter integrated manufacturing system 计算机集成制造系统bined mechanism 组合机构mon apex of cone 锥顶bine in series 串连式组合conjugate profiles共轭齿廓conjugate cam共轭凸轮connecting rod, couple 连杆cone angle 圆锥角constraint 约束constraint condition 约束条件constant acceleration and deceleration motion 等加速等减速运动规律constant diameter cam等径凸轮constant breadth cam 等宽凸轮constitution of mechanism机构组成contacting line, pressure line, line of engagement 啮合线contact ratio 重合度constant-velocity universal joints 双万向联轴节cone distance 锥距cone pulley 锥轮coordinate frame 坐标系correcting plane校正平面correcting plane 平衡平面counterweight 平衡重couple [of forces], couples 力偶couple curve 连杆曲线crank 曲柄crank-rocker mechanism曲柄摇杆机构crank angle between extreme positions极位夹角crank arm, planet carrier 系杆critical speed 临界转速circulating power load 循环功率流circular gear 圆形齿轮cross-belt drive交叉带传动crossed helical gears交错轴斜齿轮curvature曲率curved-shoe follower曲面从动件curve matching 曲线拼接cutter 刀具cycloidal gear 摆线齿轮cycloidal motion 摆线运动规律cycloidal-pin wheel 摆线针轮cycle of motion 运动周期cylindric pair 圆柱副cylindrical cam 圆柱凸轮cylindrical worm 圆柱蜗杆cylindrical coordinate manipulator 圆柱坐标操作器Ddead point 死点dedendum 齿根高dedendum circle 齿根圆degree of freedom (dof for short )自由度depth of cut 切齿深度design variable 设计变量detrimental resistance有害阻力diametral pitch 径节diametral quotient 直径系数diametral quotient 蜗杆直径系数differential gear train 差动轮系differential screw mechanism 差动螺旋机构differential screw mechanism 差动螺旋机构differentials 差速器direct (forward ) kinematics 正向运动学displacement 位移displacement diagram 位移曲线disk cam 盘形凸轮double-slider mechanism, ellipsograph 双滑块机构double crank mechanism 双曲柄机构double rocker mechanism 双摇杆机构driven pulley 从动带轮driven link, follower 从动件driven gear 从动轮driving force驱动力driving moment 驱动力矩driving link 原动件driving gear 主动齿轮driving pulley主动带轮dwell 停歇dynamic balance 动平衡dynamic balancing machine 动平衡机dynamic characteristics 动态特性dynamic reaction 动压力dynamic load 动载荷dynamic analysis of machinery机械动力分析dynamic design of machinery 机械动力设计dynamics of machinery 机械动力学Eeccentric 偏心盘effective resistance工作阻力effective resistance moment工作阻力矩end-effector 末端执行器engaging-in啮入engaging-out啮出engagement, meshing engagement, meshing 啮合epicyclic gear train 周转轮系equivalent spur gear 当量齿轮equivalent teeth number 当量齿数equivalent coefficient of friction 当量摩擦系数equivalent link 等效构件equivalent force 等效力equivalent moment 等效力矩equivalent mass 等效质量equilibrium 力平衡equivalent mechanism 替代机构equivalent moment of inertia 等效惯性力extreme position极限位置external gear 外齿轮external force 外力Fface width 齿宽face width 平底宽度feedback bining 反馈式组合field balancing 现场平衡Fifth-power polynomial motion 五次多项式运动规律final contact，end of contact 终止啮合点flat belt drive 带传动flat-face follower 平底从动件flexspline 柔轮flexible rotor 挠性转子flexible impulse, soft shock 柔性冲击flexible manufacturing system 柔性制造系统flexible automation 柔性自动化flywheel飞轮follower dwell 从动件停歇follower motion 从动件运动规律form cutting 仿形法force 力force polygon 力多边形force-closed cam mechanism 力封闭型凸轮机构forced vibration 强迫振动form-closed cam mechanism 形封闭凸轮机构four-bar linkage 四杆机构frequency of vibration 振动频率friction angle 摩擦角friction force 摩擦力friction moment 摩擦力矩friction circle 摩擦圆frame，fixed link机架frequency频率full balance of shaking force 惯性力完全平衡fundamental mechanism 基础机构fixed link, frame 固定构件function generator函数发生器Ggear 齿轮gear train 轮系gear ratio 齿数比gears 齿轮机构generating X成法generating line 发生线generating plane 发生面geneva wheel 槽轮general constraint公共约束generating line of involute 渐开线发生线generating 展成法，X成法geneva mechanism 槽轮机构governor调速器grashoff’s law 格拉晓夫定理grashoff’s law曲柄存在条件graphical design 图解设计groove cam 槽凸轮Hharmonic drive 谐波传动helical pair 螺旋副helical angle 螺旋角helix, helical line 螺旋线helical gear 斜齿圆柱齿轮herringbone gear，double helical gear 人字齿轮higher pair高副hob，hobbing cutter滚刀hob,hobbing cutter 齿轮滚刀hydrodynamic drive 液力传动hydraulic mechanism 液压机构Iimaginary part 虚部inertia force惯性力initial contact ,beginning of contact 起始啮合点inline roller follower对心滚子从动件inline flat-faced follower 对心平底从动件inline slider crank mechanism对心曲柄滑块机构input link 输入构件instantaneous center of velocity 速度瞬心instantaneous center 瞬心interchangeable gears互换性齿轮interference干涉intermittent motion mechanism 间歇运动机构internal gear 内齿轮intermittent gearing不完全齿轮inverse cam mechanism 反凸轮机构inverse (backward) kinematics 反向运动学involute 渐开线involute profile 渐开线齿廓involute gear 渐开线齿轮involute equation 渐开线方程involute function 渐开线函数involute worm 渐开线蜗杆involute helicoid 渐开线螺旋面increment or decrement work 盈亏功in-line translating follower对心移动从动件Jjacobi matrix 雅克比矩阵jerk 跃度jerk diagram 跃度曲线jointed manipulator关节型操作器Kkennedy’s theorem，theorem of three centers三心定理kinematic inversion 机架变换kinematic design of mechanism机构运动设计kinematic diagram 机构运动简图kinematic inversion 运动倒置kinematic analysis 运动分析kinematic pair 运动副kinematic diagram 运动简图kinematic chain 运动链kinematic design 运动设计kinematic synthesis 运动综合kinematic inversion 反转法knife-edge follower尖底从动件Llayout of cam profile 凸轮廓线绘制lead 导程lead angle 导程角length of contacting line 啮合线长度link 构件linkages 连杆机构line of centers 连心线load 载荷load balancing mechanism 均衡装置lower pair 低副Mmachine机器manipulator 机器人操作器machinery 机械manipulator机械手mathematical model 数学模型mass-radius product 质径积mechanism 机构mechanism机构学mechanical advantage机械利益mechanical behavior 机械特性mechanical efficiency机械效率mechanisms and machine theory, theory of mechanisms and machines机械原理mechanism with flexible elements 挠性机构meshing point 啮合点metric gears公制齿轮mid-plane 中间平面minimum teeth number 最少齿数minimum radius 最小向径module 模数modified gear 变位齿轮modification coefficient 变位系数moment 力矩moment of couple 力偶矩moment of inertia, shaking moment惯性力矩moment of flywheel 飞轮距motion skewness 运动失真moving link 运动构件Nnonstandard gear非标准齿轮noncircular gear非圆齿轮normal plane法面normal paramenters 法面参数normal circular pitch 法面齿距normal module 法面模数normal pressure angle 法面压力角nomogram诺模图number of waves 波数number of threads 蜗杆头数nut, screw nut螺母Oobjective function 目标函数offset distance 偏距offset circle 偏距圆offset roller follower 偏置滚子从动件offfser knife-edge follower 偏置尖底从动件offset flat-face follower 偏置平底从动件offset slider-crank mechanism 偏置曲柄滑块机构oldham coupling 双转块机构open-belt drive 开口传动open kinematic chain 开式链open chain mechanism 开式链机构optimal design 优化设计output work输出功output link 输出构件output mechanism 输出机构output torque 输出力矩output shaft 输出轴oscillating follower 摆动从动件oscillating guide-bar mechanism 摆动导杆机构ordinary gear train 定轴轮系other mechanism most in use 其它常用机构overlap contact ratio 纵向重合度Pparabolic motion抛物线运动partial balance of shaking force 惯性力部分平衡path generator轨迹发生器passive degree of freedom 局部自由度parallel helical gears 平行轴斜齿轮pawl 棘爪periodic speed fluctuation 周期性速度波动pinion 小齿轮pinion and rack 齿轮齿条机构pinion cutter 齿轮插刀pitch curve 理论廓线pitch point 节点pitch line节线pitch circle 节园pitch diameter节圆直径pitch cone 节圆锥pitch cone angle节圆锥角pitch curve 凸轮理论廓线planetary differential封闭差动轮系planetary drive with small teeth difference 少齿差行星传动planet gear 行星轮planet gear train行星轮系planet carrier 行星架planar pair, flat pair 平面副planar mechanism 平面机构planar kinematic pair 平面运动副planar linkage 平面连杆机构planar cam 平面凸轮pneumatic mechanism 气动机构polar coordinate manipulator球坐标操作器polynomial motion 多项式运动规律pose, position and orientation 位姿power 功率pressure angle of base circle 基圆压力角pressure angle of involute 渐开线压力角pressure angle 压力角prismatic joint 移动关节Qquick-return mechanism 急回机构quick-return characteristics 急回特性quick-return motion 急回运动Rrack 齿条rack cutter 齿条插刀radius of curvature 曲率半径radius of base circle 基圆半径radius of roller 滚子半径ratchet棘轮ratchet mechanism棘轮机构real part 实部reciprocating motion 往复移动reciprocating follower 移动从动件redundant degree of freedom 冗余自由度redundant constraint 虚约束relative velocity 相对速度relative motion 相对运动resultant force 总反力return，return-stroke 回程revolute pair 转动副revolute joint 转动关节rigid circular spline刚轮rigid impulse (shock) 刚性冲击rigid rotor 刚性转子ring gear 内齿圈rise 升程rise 推程robot 机器人robotics 机器人学robust design 稳健设计rocker 摇杆roller follower 滚子从动件roller滚子rotation guide-bar mechanism 转动导杆机构rotor with several masses 多质量转子rotating guide-bar mechanism 转动导杆机构rotor 转子round belt drive 圆带传动Sscale 比例尺screw 螺杆screw mechanism 螺旋机构self-locking 自锁shaft angle 轴角shaking couple 振动力矩simple harmonic motion (SHM for short) 简谐运动simple harmonic motion 简谐运动simple harmonic motion 余弦加速度运动sine generator, scotch yoke 正弦机构singular position 奇异位置six-bar linkage 六杆机构slider 滑块slider-crank mechanism 曲柄滑块机构sliding pair, prismatic pair移动副space 齿槽space width 齿槽宽spatial mechanism 空间机构spatial linkages 空间连杆机构spatial cams 空间凸轮机构spatial kinematic pair 空间运动副spatial kinematic chain 空间运动链speed fluctuation 速度波动spherical pair球面副spherical involute 球面渐开线spherical motion球面运动sphere-pin pair球销副spur gear 直齿圆柱齿轮starting period 起动阶段static balance 静平衡standard pitch line分度线standard pitch circle分度圆standard pitch cone分度圆锥standard spur gear 标准直齿轮steady motion period 稳定运转阶段step pulley 塔轮stopping phase 停车阶段stroke 工作行程structure 结构structural and mechanical error 结构误差sub-mechanism 子机构sun gear 太阳轮synchronous belt drive同步带传动synthesis of mechanism机构综合Ttangent mechanism正切机构teeth number 齿数tension pulley X紧轮thickness 齿厚thickness on pitch circle 节园齿厚thread pitch 螺矩thread of a screw 螺纹three-dimensional cam 三维凸轮toggle mechanism 肘形机构tooth profile 齿廓tooth curve 齿廓曲线total contact ratio 总重合度transverse plane 端面transverse parameters 端面参数transverse circular pitch 端面齿距transverse contact ratio 端面重合度transverse module 端面模数transverse pressure angle 端面压力角transmission ratio, speed ratio 传动比transmission angle 传动角two-dimensional cam 两维凸轮Uundercutting根切undercutting 过度切割universal joint 单万向联轴节unit vector 单位矢量universal joint, hooke’s coupling 万向联轴节uniform motion, constant velocity motion等速运动规律Vvelocity diagram 速度曲线vector矢量velocity 速度virtual reality 虚拟现实vibration 振动Wwave generator 波发生器wedge cam 移动凸轮width of flat-face 从动件平底宽度working space工作空间working stroke 工作行程worm 蜗杆worm gearing 蜗杆传动机构worm and worm gear 蜗杆蜗轮机构worm gear 蜗轮wrist 腕部。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

ε
θ
In TRUE BASIC， formula(公式) XB=XA+L * cos() corresponds to: XB=XA+L*COS (Q) Similarly, formula (VB)X= (VA)X -L*sin()* corresponds to VBX=VAX-L*SIN(Q)*W Formula (aB)X=(aA)X-L*sin()*-L*cos()* 2 corresponds to ABX=AAX-L*SIN(Q)*E-L*COS(Q)* W^2
3.3. Kinematic Analysis by Analytical(解析的) Methods (1) In graphical(图解的) methods, it is very tedious(冗长乏味的) if a mechanism is to be analyzed for a complete cycle. Furthermore, the accuracy(精度) is limited. (2) The analytical solution can be evaluated(求值) on a computer for different dimensions and/or at different positions with very little effort. The accuracy of the solution far surpasses(超越) that required for mechanical design problems.
ε
θ
In a Cartesian co-ordinate system(直角坐标系), ω XB=XA+L* cos(), L YB=YA+L* sin() Differentiating(求导) the above formulae(公式) with respect to time, (VB)X=(VA)X-L*sin()* (VB)Y=(VA)Y+L*cos()* Differentiating again, (aB)X=(aA)X-L*sin()*-L*cos()* 2 (aB)Y=(aA)Y+L*cos()*-L*sin()* 2
E C B A A grade II D F grade III B C C E A
g
Then the kinematic analysis of a multi-bar(多杆) linkage mechanism is reduced(简化) into two simple steps: (1)First, dividing the mechanism into Assur groups. (2)Secondly, calling(调用) the corresponding(相应的) subroutine for each Assur group according to the type and the assembly(装配) order of the Assur group.
• After the subroutine is called, (XB, YB, VBX, VBY, ABX, ABY）will be known.
3.3.2 The RRR Subroutine In the RRR group, XA , YA , (VA)X , (VA)Y , (aA)X , (aA)Y , XC , YC , (VC)X , (VC)Y , (aC)X , (aC)Y , LAB , and LCB are known. AB , AB , AB , CB , CB , CB are C to be calculated.
ε ω
L
θ
• Any subroutine must begin with statement SUB subroutine-name(table of parameters) and end with statement cilitate(使便利) the understanding of the program, parameters should have easily-recognized(认识) names. • Before the subroutine is called, （XA, YA, VAX, VAY, AAX, AAY, Q, W, E, L）are known.
Advantages of group method: Assur groups have kinematic determination. One can set up subroutines(子程序) in advance(预先) for some commonly used Assur groups.
SUB LINK(XA, YA, VAX, VAY, AAX, AAY, Q, W, E, L, XB, YB, VBX, VBY, ABX, ABY) XB=XA+L*COS(Q) YB=YA+L*SIN(Q) VBX=VAX-L*SIN(Q)*W VBY=VAY+L*COS(Q)*W ABX=AAX-L*SIN(Q)*E-L*COS(Q)*W^2 ABY=AAY+L*COS(Q)*E-L*SIN(Q)*W^2 END SUB
3.3.1 The LINK Subroutine Suppose that the X and Y components(分量) of position, velocity, and acceleration of a point A (i.e. XA, YA, (VA)X, (VA)Y, (aA)X, (aA)Y), the angular position, the angular velocity, and the angular acceleration of link AB(i.e. , , ), and the length (L) of the link AB are known. XB, YB, (VB)X, (VB)Y, (aB)X, (aB)Y are to be calculated. ω L