北大计量经济学讲义-工具变量与两阶段最小二乘法
工具变量估计与两阶段最小二乘法

2 0.014 n 428, R 0.118
edu 10.24 0.269 fatheduc n 428, R 2 0.173 n 428, R 2 0.093 log wage 0.441 0.059educ
0.446 0.035
ˆ u2 SSTx Rx2, z
ˆ 的方差越小; n,或 ,或 越大, 1
2 x 2 x,z
在高斯-马尔科夫假定下,OLS估计量的方差:
ˆ Var 1 SSTx
2
例1 估计已婚女性的教育回报
log wage 0.185 0.109educ
0.185 0.28 0.029
IV 0.132 (0.055) 0.108 (0.024) -0.0023 (0.0003)
Black
Smsa South 观测数 R2
-0.199 (0.018)
0.136 (0.02) -0.148 (0.026) 3010 0.300
-0.147 (0.054)
ˆ ˆ y ˆ z 0 1 i 2 2 i1 0
i1
z y
i 1 i2
ˆ ˆ y ˆ z 0 1 i 2 2 i1 0 ˆ ˆ y ˆ z 0 1 i 2 2 i1 0
z y
北大计量经济学讲义-工具变量与两阶段最小二乘法

large numbers. 当假定(15.4) 和(15.5) 成立时,可以应用大
数定律证明IV估计是b1的一致估计。
Intermediate Econometrics,
That is, Cov(z,u) = 0 (15.4) 即Cov(z,u) = 0
Intermediate Econometrics,
Yan Shen
8
Instrumental Variable: Who qualifies? 什么样的变量可以作为IV?
The instrument must be correlated with the endogenous variable x 工具变量应与内生变量 x 相关
Intermediate Econometrics,
Yan Shen
5
Why Use Instrumental Variables? 为何使用工具变量?
Instrumental Variables (IV) estimation is used when your model has endogenous x’s 当模型解释变量具有内生性时,使用工具 变量估计
Suppose the true model regresses log(wage) on education (educ) and ability (abil). 假定真实模型将对数工资对教育和能力回归
Now ability is unobserved, and the proxy, IQ, is not available. 现在能力不可观测,而且没有代理变量IQ
b1 . 当z=x时,我们得到b1的OLS估计
第15章 工具变量估计与两阶段最小二乘法

Eu 0, Ez1u 0,L , E zk1u 0, E zku 0
15.3 两阶段最小二乘法
如果一个内生解释变量有多个工具变量,如 何有效运用多个工具变量?以下面结构模 型为例: y1 0 1y2 2z1 u1
则称z是x的工具变量(IV)。工具变量可得 能够用于一致估计回归方程的参数。
15.1 动机:简单回归模型中的遗漏变量
借助于工具变量,回归方程的参数是可识别 (identification),即参数可用总体矩表示, 而总体矩可用样本矩来估计。用工具变量z 对回归方程两边协方差:
cov z, y 1 cov z, x cov z,u
对此也没有合理的解释。
15.2 多元回归模型中的IV估计
简单回归模型IV估计很容易延伸到多元回归
y1 0 1 y2 2 z1 L k zk1 u1
借用联立方程模型的形式和术语,此方程称 为结构方程(structural equation)。 z1, z2 ,L , zk1是外生变量,y2 被怀疑是内生的, 即可能与u相关。需要找到其工具变量
有效的工具变量 zk 需满足:(1)是未包含的 外生变量,即它不在结构方程中且与u不相 关。
15.2 多元回归模型中的IV估计
(2)zk 与 y2 存在某种偏相关,即约简型方程
y2 0 1z1 L k1zk1 k zk v
的系数满足: k 0
同样要求(1)不能检验,只能寄希望于经济 逻辑和反思。要求(2)可对约简型方程估 计后直接检验。
第十五章 工具变量估计与两阶段 最小二乘法
本章研究多元回归模型中的内生解释变 量问题。解释变量的内生性常来自遗漏 变量问题,这使OLS估计不一致。尽管 代理变量法和面板数据的固定效应法可 在某些情况下解决内生性问题,但远未 完善。本章讨论另一解决解释变量内生 性问题的方法:工具变量法(IV)。其 受欢迎程度仅次于OLS。
第15章-工具变量讲解

这样一来 , 我们便把 abil 放人误差项中,而 只留下简单回归模型: Log(wage) =β 0+β 1educ+u (15.1 ) 其中,u 包含 abil。当然,如果用 OLS 估计 方程 (15.1) ,若 educ 与 abil 相关,则得到 的结果将是 1 的有偏而又不一致估计量。
第15章 工具变量估计与两阶段最小二乘法
在本章中,我们进一步研究多元回归模型中的 内生解释变量 (endogenous explanatory Variables) 问题。在第 3 章中,我们推导出遗漏一个重要变 量时 OLS 估计量的偏误,在第 5 章中,我们说明 了在遗漏变量(omitted variables)的情况下,OLS 通 常是不一致的。
举例来说,考虑成年劳动者的工资方程中存 在无法观测之能力因素的问题。一个简单的 模型为: log(wage)=β 0+β 1educ+β 2abil+e 其中,e 是误差项。
在第 9 章中,我们证明了在某些假定下,如 何用诸如 IQ 的代理变量代替能力,从而通过 以下回归可得到一致估计量 log(wage)对 educ,IQ 回归 然而假定不能得到适当的代理变量(或它不 具备足以获取 1 一致估计量所需的性质)。
我们一开始先说明,在存在遗漏变量的情况 下,如何用 IV 法获得一致估计量。此外, IV 至少能在某些假定下用于解决变量误差 (errors-in-variables)问题。下一章将证明运 用 IV 法如何估计联立方程模型。
我们对工具变量估计的论述严格遵照我们在 第 1 篇中对普通最小二乘的推导,其中假定 我们有一个来自潜在总体的随机样本。这个 起点很合人意,因为除了简化符号之外,它 还强调用潜在总体来表述对 IV 估计所做的重 要假定 (正如用 OLS 时一样)。
两阶段最小二乘法步骤

两阶段最小二乘法步骤
两阶段最小二乘法是一种分离策略,将内生变量分离为可以被工具变量线性表出的部分,以及随机干扰部分。
其具体步骤如下:
1. 第一阶段:让工具变量z对内生x进行回归,得到估计值$x^$。
2. 第二阶段:利用$x^$对y做回归,得到系数估计值。
这种方法通过将估计分成两个步骤(阶段)回归,因此得名“两阶段最小二乘法”。
对于联立方程组,可以采用三阶段最小二乘法。
如果存在弱工具变量问题,可以采取对信息不太敏感的有限信息极大似然估计法。
c15 工具变量估计与两阶段最小二乘法

第15章工具变量估计与两阶段最小二乘法摘要: 本章继续讨论如何解决模型中的内生解释变量(endogenous explanatory variables )问题。
遗漏变量(omitted variables )是导致内生性问题的一个原因。
本章采用工具变量法(method of instrumental variables,IV )来解决模型中的一个或多个解释变量的内生性问题。
所采用的估计方法被称为两阶段最小二乘估计(method of two stage least squares ,2SLS or TSLS),其受欢迎程度仅次于OLS. IV 也能在某些特定的情形下解决变量带误差(errorsin-variables )的问题.15.1 动机: 简单回归中的遗漏变量如何处理可能发生的变量遗漏带来的偏误,已有三种选择: 1)直接忽略,讨论偏误的方向;2)寻找一个合适的代理变量;3)如果该遗漏变量不随时间变化时,采用FE 或FD 方法。
工具变量法的思路:不是考虑如何处理遗漏变量(此时遗漏变量在误差项中),而是寻找被遗漏的解释变量的替代变量,使得替代变量和误差项不再存在相关性。
y =β0+β1x +u ,此时该模型不满足MLR.4,从而不能保证Cov (x,u )=0,特别地,假定Cov (x,u )≠0. 如果x 的替代变量z 同时满足下面两个条件:1) 工具外生性(instrument exogeneity )条件:Cov (z,u )=0,2) 工具相关性(instrument relevance )条件:Cov (z,x )≠0,则称z 为x 的工具变量(instrumental variable ),或简称工具(instrumental ). 几点说明:1) 工具变量的外生性意味着z 对y 没有偏效应(当x 和u 中遗漏变量被控制时),同时也和其它被遗漏变量不相关;2) 工具外生性检验在多数情况下只能通过经济行为或反思来判断;3) 工具相关性检验借助t 和F 检验就行;外生性和相关性假设足以帮助我们识别(Identification )出β1=COv(z,y)Cov(z,x),那么β1的工具变量估计(instrumental variables (IV) estimator )为:β̂1=∑(z i −z ̅)(y i −y ̅)n i=1∑(z i −z ̅)(x i −x ̅)n i=1, 其是β1的一致但有偏的估计;4)β̂1显然当z=x,该估计就是OLS 估计,但这要以x 和u 无关为条件,也即工具变量法适于u 和x 无关的情形。
工具变量两阶段最小二乘

两阶段最小二乘法:TSLS
点击选择按钮(Op>ons)对参数估计协方差矩 阵的估计方法进行选择,本例采用的是横截面数据, 因此采用怀特异方差一致的协方差矩阵估计。
6.2 工具变量估计方法
6.2.2 两阶段最小二乘法:TSLS
两阶段最小二乘法:TSLS
一个内生自变量
Y = β0 + β1X1 + β2 X 2 + β3 X3 + u X1 为内生变量, X2 和X 3 为外生变量,Z1 、Z2 X为1 的工具变量。 两阶段最小二乘步骤:
原假设: H0 : α1 = α2 = 0
• 用第五章构造的Tr 统计量进行F检验,若 Tr值够大, 通常大于10则认为相关性足够,可做工具变量。
• 若接受原假设,则表明工具变量与内生变量相关 性太弱,其不适宜做工具
两阶段最小二乘法:TSLS
一个内生自变量
EViews实现两阶段最小二乘: 例子6.2 已婚女性小时工资(续)
• 不相干变量引入不会影响参数估计的无偏性和一 致性,但是会影响参数估计的有效性。
6.2 工具变量估计方法
6.2.1 工具变量估计法 6.2.2 两阶段最小二乘法:TSLS
6.2 工具变量估计方法
6.2.1 工具变量估计法
工具变量估计法
一元线性回归模型
Y = β0 + β1X + u
Ø 定义1:如果存在变量Z
工具变量法与最小二乘法的联系

工具变量法与最小二乘法的联系引言在经济学研究中,经常会遇到因果关系的分析问题。
然而,由于一些内生性问题,经济变量之间的因果关系不容易准确确定。
在这种情况下,研究者常常会使用工具变量法来解决内生性问题。
而在回归分析中,最小二乘法是最常用的估计方法之一。
本文将讨论工具变量法与最小二乘法的联系,并探讨它们在经济研究中的应用。
第一节:最小二乘法的基本原理最小二乘法是回归分析中最常用的估计方法之一。
其基本思想是通过最小化实际观测值与拟合值之间的残差平方和,来估计模型参数。
最小二乘法在非内生性问题下具有较好的性质和可解释性,因此被广泛应用于经济学研究。
第二节:工具变量法的基本原理工具变量法是一种解决内生性问题的方法。
当存在内生性问题时,直接使用最小二乘法估计结果可能是无偏且一致的,但标准误差可能会被低估,导致统计显著性的判断错误。
工具变量法通过引入一个或多个与内生变量相关但与误差项不相关的工具变量,将内生变量的影响通过工具变量间接传递给被解释变量,从而实现对内生性问题的处理。
第三节:虽然最小二乘法和工具变量法在解决经济研究中的问题时采用不同的方法,但它们之间存在联系。
首先,最小二乘法可以视为工具变量法的一种特殊情况,在非内生时可以直接使用。
其次,最小二乘法可以通过工具变量法来解决内生性问题,从而得到更准确的估计结果。
工具变量法通过引入工具变量来处理内生性问题,而这些工具变量的选择和使用通常需要基于最小二乘法的思想。
例如,研究者可以利用工具变量与内生变量相关的结构特点,通过最小二乘法来选择合适的工具变量。
这种联系使得最小二乘法和工具变量法之间相辅相成,共同构建了解决内生性问题的分析框架。
第四节:工具变量法与最小二乘法的应用工具变量法和最小二乘法在实际应用中都非常重要。
最小二乘法常被用于线性回归分析,估计参数的一致性和渐进正态性。
而工具变量法则广泛应用于处理内生性问题,如评估教育对收入的影响、估计负债对企业投资决策的影响等。
两阶段最小二乘法定义

两阶段最小二乘法定义“同学们,今天咱们来聊聊两阶段最小二乘法。
”我站在讲台上,看着下面一双双充满求知欲的眼睛说道。
那什么是两阶段最小二乘法呢?简单来说,它是一种用于解决内生性问题的计量经济学方法。
比如说,我们想研究教育程度对收入的影响,但可能存在一些其他因素,既影响教育程度又影响收入,这就是内生性问题。
举个例子吧,假设我们要研究一个地区的经济发展水平和环境污染之间的关系。
经济发展水平可能是内生变量,因为可能有一些其他未被观察到的因素,既影响经济发展又影响环境污染。
如果我们直接用普通最小二乘法去估计,可能会得到有偏差的结果。
两阶段最小二乘法就分两个阶段来处理这个问题。
在第一阶段,我们找到一个工具变量,这个工具变量要和内生变量相关,但又不能直接影响因变量。
然后用这个工具变量去预测内生变量。
在第二阶段,我们把第一阶段预测得到的内生变量的值代入到模型中,用普通最小二乘法进行估计。
比如说,在刚才那个例子中,我们可以找一个和经济发展水平相关的工具变量,比如这个地区的基础设施建设情况。
基础设施建设好可能会促进经济发展,但它对环境污染的直接影响相对较小。
在第一阶段,我们用基础设施建设情况去预测经济发展水平,得到预测值。
在第二阶段,我们把这个预测值代入到模型中,来估计经济发展水平对环境污染的影响。
两阶段最小二乘法的优点就在于它能有效地处理内生性问题,让我们得到更可靠的估计结果。
但是它也不是完美的,它对工具变量的要求比较高,如果工具变量选择不当,可能会导致估计结果更糟糕。
再给大家说个实际的例子,有研究想知道广告投入对产品销量的影响。
但可能存在一些其他因素,比如市场趋势、季节变化等,既影响广告投入又影响销量。
这时候就可以用两阶段最小二乘法。
找到一个合适的工具变量,比如同行业其他公司的广告投入情况,来解决内生性问题。
同学们,希望通过今天的讲解,大家对两阶段最小二乘法有了更清楚的认识和理解。
以后在遇到类似问题的时候,能想到用这种方法来解决。
伍德里奇《计量经济学导论》(第5版)笔记和课后习题详解-第15章工具变量估计与两阶段最小二乘法【圣

伍德里奇《计量经济学导论》(第5版)笔记和课后习题详解-第15章工具变量估计与两阶段最小二乘法【圣第15章工具变量估计与两阶段最小二乘法15.1复习笔记一、动机:简单回归模型中的遗漏变量1.面对可能发生的遗漏变量偏误(或无法观测异质性)的四种选择(1)忽略遗漏变量问题,承受有偏而又不一致估计量,若能把估计值与关键参数的偏误方向一同给出,则该方法便令人满意。
(2)试图为无法观测变量寻找并使用一个适宜的代理变量,该方法试图通过用代理变量取代无法观测变量来解决遗漏变量的问题,但并不是总可以找到一个好的代理。
(3)假定遗漏变量不随时间变化,运用固定效应或一阶差分方法。
(4)将无法观测变量留在误差项中,但不是用OLS 估计模型,而是运用一种承认存在遗漏变量的估计方法,工具变量法。
2.工具变量法简单回归模型01y x uββ=++其中x 与u 相关:()Cov 0,x u ≠(1)为了在x 和u 相关时得到0β和1β的一致估计量,需要有一个可观测到的变量z,z 满足两个假定:①z 与u 不相关,即Cov(z,u)=0;②z 与x 相关,即Cov(z,x)≠0。
满足这两个条件,则z 称为x 的工具变量,简称为x 的工具。
z 满足①式称为工具外生性条件,工具外生性意味着,z 应当对y 无偏效应(一旦x 和u 中的遗漏变量被控制),也不应当与其他影响y 的无法观测因素相关。
z 满足②式意味着z 必然与内生解释变量x 有着或正或负的关系。
这个条件被称为工具相关性。
(2)工具变量的两个要求之间的差别①Cov(z,u)是z 与无法观测误差u 的协方差,通常无法对它进行检验:在绝大多数情形中,必须借助于经济行为或反思来维持这一假定。
②给定一个来自总体的随机样本,z 与x(在总体中)相关的条件则可加以检验。
最容易的方法是估计一个x 与z 之间的简单回归。
在总体中,有01x z vππ=++从而,由于()()1Cov /ar V ,x z z π=所以式Cov(z,x)≠0中的假定当且仅当10π≠时成立。
工具变量法IV两阶段最小二乘法TSLS

原解释变量 X y2, y3,L , ym1, xk1,L , xk
Dongbei University Of Finance & Economics
三者之间的关系
ILS是TSLS的一种特殊形式,而ILS和TSLS都 是工具变量法。即有:
系数矩阵为:
1 b12 0 c11 c12 0
,
0
1 b23 0
0
c23
b31 b32 1 0
0 c33
Dongbei University Of Finance & Economics
计算出1,得到简化式参数 1,从而 得到模型简化式:
2)大样本下的TSLS估计量是一致的;
3)方程恰好识别时,ILS与TSLS估计一致;
4)模型可识别时,每一个结构方程都可用TSLS估计参数。
TSLS是最常用的方法——先建立理论联立结构方程组模型,再 进行单个方程的TSLS估计。
四、工具变量法IV
ILS和TSLS都属于工具变量法。工具变量法,
即对模型方程中出现随机解释变量X时,选择一个
模型可写成矩阵形式:
Y1 b12Y2 0Y3 c11 X1 c12 X 2 0 X 3 u1 0Y1 Y2 b23Y3 0 X1 0 X 2 c23 X 3 u2 b31Y1 b32Y2 Y3 0 X1 0 X 2 c33 X 3 u3
• 二、对简约式模型逐个方程求OLS,得到简约式 模型中所有的参数估计值;
• 三、将简约式参数估计值代入关系式,求结构方 程参数的估计值。
第15章-工具变量与两阶段最小二乘知识分享

第15章 工具变量估计与两阶段最小二乘法在本章中,我们进一步研究多元回归模型中的内生解释变量(endogenous explanatory variable )问题。
在第3章中,我们推导出,遗漏一个重要变量时OLS 估计量的偏误;在第5章中,我们说明了在遗漏变量(omitted variable )的情况下,OLS 通常是非一致性的。
第9章则证明了,对未观测到的解释变量给出适宜的代理变量,能消除(或至少减轻)遗漏变量偏误。
不幸的是,我们不是总能得到适宜的代理变量。
在前两章中,我们解释了存在不随时间变化的遗漏变量的情况下,对综列数据如何用固定效应估计或一阶差分来估计随时间变化的自变量的影响。
尽管这些方法非常有用,可我们不是总能获得综列数据的。
即使能获得,如果我们的兴趣在于变量的影响,而该变量不随时间变化,它对于我们也几无用处:一阶差分或固定效应估计排除了不随时间变化的变量。
此外,迄今为止我们已研究出的综列数据法还不能解决与解释变量相关的随时间而变化的遗漏变量的问题。
在本章中,我们对内生性问题采用了一个不同的方法。
你将看到如何用工具变量法(IV )来解决一个或多个解释变量的内生性问题。
就应用计量经济学中线性方程的估计而言,两阶段最小二乘法(2SLS 或TSLS )是第二受人欢迎的,仅次于普通最小二乘。
我们一开始先说明,在存在遗漏变量的情况下,如何用IV 法来获得一致性估计量。
此外,IV 能用于解决含误差变量(errors-in-variable )的问题,至少是在某些假定下。
下一章将证明运用IV 法如何估计联立方程模型。
我们对工具变量估计的论述严格遵照我们在第1篇中对普通最小二乘的推导,其中假定我们有一个来自基本总体的随机样本。
这个起点很合人意,因为除了简化符号之外,它还强调了应根据基本总体来表述对IV 估计所做的重要的假定(正如用OLS 时一样)。
如我们在第2篇中所示,OLS 可以应用于时间序列数据,而工具变量法也一样可以。
第15章 工具变量估计与两阶段最小二乘法

Eu 0, Ez1u 0,L , E zk1u 0, E zku 0
15.3 两阶段最小二乘法
如果一个内生解释变量有多个工具变量,如 何有效运用多个工具变量?以下面结构模 型为例: y1 0 1y2 2z1 u1
如果内生解释变量 y2有两个被排斥的外生变 量 z2 , z3,且都与 y2相关,则不仅其中任何 一个可作为IV,而且它们的任何线性组合也 是有效的IV。为了找到最好的IV,需选择与 y2
最高度相关的线性组合,这要求估计约简型 方程: y2 0 1z1 2 z2 3z3 v2
第十五章 工具变量估计与两阶段 最小二乘法
本章研究多元回归模型中的内生解释变 量问题。解释变量的内生性常来自遗漏 变量问题,这使OLS估计不一致。尽管 代理变量法和面板数据的固定效应法可 在某些情况下解决内生性问题,但远未 完善。本章讨论另一解决解释变量内生 性问题的方法:工具变量法(IV)。其 受欢迎程度仅次于OLS。
大样本下IV估计量近似服从正态分布,常用的推断 方法均适用,但IV估计的方差总是大于OLS方差, 特别当z与x的线性相关度很弱时,IV方差会比 OLS方差大很多。由此当x外生时,OLS估计比IV 估计有效得多。
IV估计的关键是找到好的工具变量,工具变量的两 个要求具有很大差别,要求(1)涉及无法观测的 误差项,通常无法对其进行检验,在绝大多数情 况下,我们必须借助于经济行为或反思来维持此 假定。
15.3 两阶段最小二乘法
在对 2 , 3 的联合显著性检验之后,使用估计值作
为 y2的IV: yˆ2 ˆ0 ˆ1z1 ˆ2 z2 ˆ3 z3
在多个工具条件下IV估计量称为两阶段最小二乘估 计量(2SLS)。原因是当用以上估计值作为IV时, 参数的IV估计值等同于 y1 yˆ2 , z1回归的OLS估 计值。但标准误和检验统计量不相同。
伍德里奇《计量经济学导论》(第6版)复习笔记和课后习题详解-工具变量估计与两阶段最小二乘法

第15章工具变量估计与两阶段最小二乘法15.1复习笔记考点一:工具变量法★★★★★1.简单模型的工具变量法简单回归模型为y=β0+β1x+u,其中x与u相关:Cov(x,u)≠0。
(1)为了在x和u相关时得到β0和β1的一致估计量,需要有一个可观测到的变量z,z满足两个假定:①工具外生性条件,z与u不相关,即Cov(z,u)=0,意味着z应当对y无偏效应(一旦x和u中的遗漏变量被控制),也不应当与其他影响y的无法观测因素相关;②工具相关性条件,z与x相关,即Cov(z,x)≠0,意味着z必然与内生解释变量x 有着或正或负的关系。
满足这两个条件,则z称为x的工具变量,简称为x的工具。
(2)工具变量的两个要求之间的差别①Cov(z,u)是z与无法观测误差u的协方差,通常无法对它进行检验:在绝大多数情形中,必须借助于经济行为或反思来维持这一假定。
②给定一个来自总体的随机样本,z与x(在总体中)相关的条件则可加以检验。
最容易的方法是估计一个x与z之间的简单回归。
在总体中,有x=π0+π1z+v,从而,由于π1=Cov(z,x)/Var(z)所以式Cov(z,x)≠0中的假定当且仅当π1≠0时成立。
因而就能够在充分小的显著水平上,相对双侧对立假设H 1:π1≠0而拒绝虚拟假设H 0:π1=0。
就能相当有把握地肯定工具z 与x 是相关的。
2.工具变量估计量(1)参数的工具变量(IV)估计量参数的识别意味着可以根据总体矩写出β1,而总体矩可用样本数据进行估计。
为了根据总体协方差写出β1,利用简单回归方程可得z 与y 之间的协方差为:Cov(z,y)=β1Cov(z,x)+Cov(z,u)在Cov(z,u)=0与Cov(z,x)≠0的假定下,可以解出β1为:β1=Cov(z,y)/Cov(z,x)β1是z 和y 之间的总体协方差除以z 和x 之间的总体协方差,说明β1被识别了。
给定一个随机样本,用对应样本量来估计总体量。
北大计量经济学讲义-第二讲
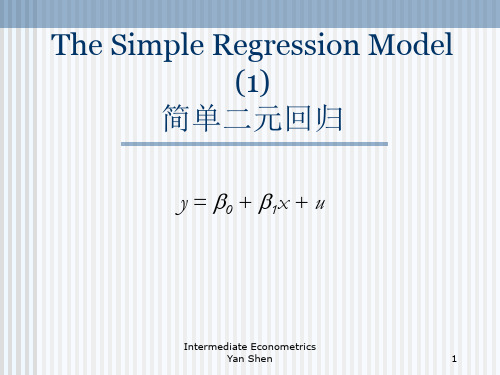
Intermediate Econometrics Yan Shen
14
Zero Conditional Mean Assumption
条件期望零值假定
In the example of education, suppose u represents innate ability, zero conditional mean assumption means E(ability|edu=6)=E(ability|edu=18)=0. The average level of ability is the same regardless of years of education. 在教育一例中,假定u 代表内在能力,条件期望 零值假定说明不管解释教育的年限如何,该能力 的平均值相同。
Intermediate Econometrics Yan Shen 6
Some Terminology
术语注解
The coefficients b0 , b1 are called the regression coefficients. b0 is also called the constant term or the intercept term, or intercept parameter. b1 represents the marginal effects of the regressor, x. It is also called the slope parameter. b0 , b1被称为回归系数。 b0也被称为常数项或截矩 项,或截矩参数。 b1代表了回归元x的边际效果, 也被成为斜率参数。
Dependent Variable, or Left-Hand Side Variable, or Explained Variable, or Regressand 在简单二元回归模型y = b0 + b1x + u中, y通常被称为 因变量,左边变量,被解释变量,或回归子。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
nehS naY ,scirtemonocE etaidemretnI
计估SLO的1b到得们我�时x=z当 . 1b
计估�时在存VI当 noitamitsE :elbaliavA si VI na nehW
91
nehS naY ,scirtemonocE etaidemretnI
计估�时在存VI当 noitamitsE :elbaliavA si VI na nehW
�量变具工用使何为 ?selbairaV latnemurtsnI esU yhW
7
nehS naY ,scirtemonocE etaidemretnI
题问差误量测的典经决解来用可VI�且而 melborp selbairav-ni-srorre cissalc eht evlos ot desu eb nac VI ,yllanoitiddA � 差偏量变漏遗决解来用以可VI�以所 saib elbairav dettimo fo melborp eht sserdda ot desu eb nac VI ,suhT �
�
定决资工�子例 noitanimreted egaw :elpmaxE
41
nehS naY ,scirtemonocE etaidemretnI
。关相项差误和育教与时同它。不 .mret rorre eht dna noitacude htob htiw setalerroc tI .oN � �吗量变具工的好是QI ?tnemurtsni doog a QI sI �
。计估致一的1b是计估VI明证律定数 大用应以可�时立成 )5.51(和 )4.51(定假当 .srebmun egral fo wal eht gniylppa retfa ,1b rof tnetsisnoc si rotamitse VI eht taht wohs nac eno ,dloh )5.51( dna )4.51( snoitpmussa nehW �
�
定决资工�子例 noitanimreted egaw :elpmaxE
31
nehS naY ,scirtemonocE etaidemretnI
�
。关相不项差 误与且并�关相度高平水育教与当应VI的好个一 .mret rorre eht htiw detalerroc ton tub ,noitacude htiw detalerroc ylhgih eb ot deen VI doog A 。题问性生内的育教现出会时此�关相 力能与平水育教且并�QI含包项差误于由�题问 .sraeppa melborp ytienegodne ,QI htiw setalerroc noitacude dna ,QI sniatnoc mret rorre eht ecnis :melborP
� x � ix�� z � iz� � � 1b � y � iy �� z � iz� � ˆ
81
。等恒计估SLO与计估VI的时这�VI 的己自作它用以可�时生外是x当着味意这 .esac siht ni SLO ot lacitnedi si rotamitse VI eht dna ,VI nwo sti sa desu eb nac ti ,suonegoxe si x nehw snaem sihT � fo rotamitse SLO eht niatbo ew x=z nehW �
)u,z(voC于关 )u,z(voC tuobA
11
nehS naY ,scirtemonocE etaidemretnI
。归回段阶一第为称归回个这将们我时有 .noisserger egats-tsrif eht sa noisserger siht ot refer ew semitemoS � v + z1p + 0p = x ni 0 = 1p :0H 验检需只 v + z1p + 0p = x ni 0 = 1p :0H gnitset tsuJ � 0 ≠ )x,z(voC否是验检以可们我 0 ≠ )x,z(voC fi tset nac eW �
)x,z(voC tuobA
21
nehS naY ,scirtemonocE etaidemretnI
�
�
归回育教对资工数对将�归回的用使上实事 .cude no )egaw(gol sserger :noisserger lautca ehT QI量变理代有没且而�测观可不力能在现 .elbaliava ton si ,QI ,yxorp eht dna ,devresbonu si ytiliba woN 归回力能和育教对资工数对将型模实真定假 .)liba( ytiliba dna )cude( noitacude no )egaw(gol sesserger ledom eurt eht esoppuS
定决资工�子例 noitanimreted egaw :elpmaxE
51
nehS naY ,scirtBiblioteka monocE etaidemretnI
�
� �
。的关相不力 能与是们它定肯要需都们我�VI为作个一哪的中其用使们我论无 .ytiliba htiw detalerroc ton era yeht taht tnedifnoc eb ot deen ew ,VI sa meht fo rehtie esu ot htiw ew fI 低越平水育教受均平�多越妹姐弟兄�说假。目数妹姐弟兄 .noitacude fo slevel egareva rewol htiw detaicossa si sgnilbis erom :sisehtopyH .sgnilbis fo rebmuN 平水育教亲母 noitacude s’rehtoM
5
nehS naY ,scirtemonocE etaidemretnI
� �
。法方计估的健稳量变漏遗对种一用使 elbairav dettimo eht fo ecneserp eht sezingocer taht dohtem noitamitse na sesU 量变理代用使 yxorp elbatius a esu dna dniF 在存不并题问个这装假�题问个这略忽 tsixe ton seod ti taht dneterp ,melborp eht erongI
要提课本 eniltuO erutceL
4
nehS naY ,scirtemonocE etaidemretnI
�办么 怎当应们我�漏遗被量变的要重些一果如 ?od ew dluohs tahw ,dettimo era selbairav tnatropmi fI �
…发出题问个这从 htiw trats ot melborP
要提章本 eniltuO retpahC
3
nehS naY ,scirtemonocE etaidemretnI
� � � � �
计估VI的归回程方多 ledom noisserger elpitlum eht fo noitamitse VI 方R的计估VI算计 VI retfa serauqs R gnitupmoC 质性的量变具工”坏― VI roop a htiw VI fo seitreporP 断推计统的中计估 VI rotamitse VI eht htiw ecnerefnI lacitsitatS �量变具工用何为�点发出 ?VI gnisu yhW :noitavitoM
�
�VI为作以可量变的样么什 ?seifilauq ohW :elbairaV latnemurtsnI
9
nehS naY ,scirtemonocE etaidemretnI
�VI为作以可量变的样么什 ?seifilauq ohW :elbairaV latnemurtsnI
)5.51( 0 ≠ )x,z(voC ,si tahT � 关相 x 量变生内与应量变具工 x elbairav suonegodne eht htiw detalerroc eb tsum tnemurtsni ehT �
�
法办些一 tuo syaw ehT
6
nehS naY ,scirtemonocE etaidemretnI
时0≠ )u,x(voC�即 0 ≠ )u,x(voC nehw ,si tahT � 计估量变 具工用使�时性生内有具量变释解型模当 s’x suonegodne sah ledom ruoy nehw desu si noitamitse )VI( selbairaV latnemurtsnI �
2
nehS naY ,scirtemonocE etaidemretnI
� � � � �
性生内验检 …ytienegodne rof gnitseT 题问差误量测决解量变具工用 melborp selbairav-ni-srorre ot snoitulos VI 法乘二小最段阶两 serauqS tsaeL egatS owT 计估量变具工的中归回程方多 noissergeR elpitluM eht fo noitamitse VI 量变漏遗的中归回单简 ledom noisserger elpmis a ni selbairaV dettimO
01
nehS naY ,scirtemonocE etaidemretnI
。论理济经和识常于赖依 不得不们我 �理合否是定假一这0 = )u,z(voC断判了为 0 = )u,z(voC emussa ot esnes sekam ti fi ediced ot yroeht cimonoce dna esnes nommoc esu ot evah eW �
1
nehS naY ,scirtemonocE etaidemretnI
v + kxkp . . . + 2x2p + z1p + 0p = 1x u + kxkb . . . + 2x2b + 1x1b + 0b = y
