AP微积分BC公式大全
ap微积分公式

AP微积分(Pre-calculus)公式大全三角函数(trigonometric function)三角函数值图像变换(transfermation)三角函数公式(identity)反三角函数(inverse trigonometric function)正弦定理与余弦定理(law of sine and cosine)三角形面积公式Heron’s formula解析几何(analytic geometry)直线(line)斜率(slope)平行:斜率相等垂直:斜率相乘等于-1两点间的距离公式(distance)点到直线的距离公式中点公式(midpoint)定比分点公式抛物线(parabola)圆锥曲线(conics)圆(circle)椭圆(ellipse)双曲线(hyperbola)抛物线(parabola)图像旋转(rotation)空间解析几何两点距离公式(distance between two points)中点坐标公式(midpoint)平面(plane)球(sphere)向量(vector)运算(operation)投影(projection)空间向量(vector in space)极坐标(polar)常见极坐标方程(polar equation)复数(complex number)运算(operation)直角坐标形式(rectangular form)极坐标形式(polar form)矩阵(matrix)数列(sequence)概率统计(probability and statistics)排列组合(permutation and combination)概率(probability)二项式定理(Binomial theorem)。
AP微积分-AP Calculus 公式大全-217

AP Calculus BC1. Important limits()001111011sin sin lim1, lim 1lim 1lim 10, ()lim lim , x x xt x t m m m m m m n n x x x ax ax bx be t e x m n P x a x a x a x a a m n b x b →→→∞→---→∞→∞==⎛⎫+=⇔+= ⎪⎝⎭<++++===+++⎧⎪⎪⎨x x sec )'(tan = x csc (cot)'-= x x x tan sec )'(sec = x x x cot csc )'(csc -=(6) 211)'(arcsin xx -=211)'(arccos xx --=211)'(arctan x x +=211)'cot (x x ar +-= 11)'sec (2-=x x x arc 11)'csc (2--=x x x arc2. Rules(1)If f (x ),g (x ) are differential ,a. )()())()((x g x f x g x f '±'='±;b. )()()()())()((x g x f x g x f x g x f '+'=',especially ,)())((x f C x Cf '='(C is a constant );c. )0)(( ,)()()()()())()((2≠'-'='x g x g x g x f x g x f x g x f ,especially ,21()()()()g x g x g x ''=-。
(2) Chain Rule )]([x g f y =⇒dxdudu dy dx dy ⋅= (3) Implicit Differentiation ()(()'r t f =3. Applications of Derivative Mean Value Theorem) is differentiable Tangent and normalFirst Derivative Test'f changes from - to +,local min 'f changes from + to -,local maxSecond Derivative Test ''()0f a >,local min ''()0f a <,local max(4) Absolute max/min: compare function value of critical point and endpoints.Steps: 1. find 'f ;2.solve '0f =;3. compare value at critical point and endpoints.1. Indefinite Integral:'()()Definition f x dx f x C =+⎰ Method ()()df x f x C =+⎰2. Methods for Indefinite Integral 不定积分方法 ① Formulas :dx x C =+⎰(integrand 为1), kdx kx C =+⎰, 11n nx x dx C n +=++⎰ln x xa a dx C a=+⎰ x xe dx e C =+⎰1sin 2 4x x C ++②()f u du3. Find the antiderivative of ()f u .③ Partial fraction (拆分)ln ||ln ||()()cx d A Bdx dx dx A x a B x b C x a x b x a x b +=+=-+-+----⎰⎰⎰(通分求A,B)④ Integration by partsudv uv vdu =-⎰⎰,(or ''uv dx uv vu dx =-⎰⎰), Tabular Integration , (story about ln x , sin x , and xe )3. Improper Integral 反常积分(两种形式,无穷积分,瑕积分) ① Integral on infinite interval()lim (),()lim (),()()()lim ()lim ().ba ab bbaa c cbcaca b f x dx f x dx f x dx f x dx f x dx f x dx f x dx f x dx f x dx ∞→∞-∞→-∞∞∞-∞-∞→-∞→∞===+=+⎰⎰⎰⎰⎰⎰⎰⎰⎰② Integrand with infinite discontinuities()lim (),bca ac bbbf x dx f x dx -→=⎰⎰4.5. )'()x β⋅6. ①(,)c a b ∈. ②low upper function er function a⎣⎦L Right functio ef func on n ti )cy dy ⎤⎥⎣⎦③ V olume :with known cross section()baA x dx ⎰, ()A x is the area of the cross section.Revolution :2Inner 2Out rad er radiu ius s baR r dx π⎡⎤-⎣⎦⎰,Shell Method :2baxydx π⎰(旋转轴为y-axis ) or 2baxydy π⎰(旋转轴为x-axis )④ Length21x x ⎰or 21y y ⎰or 21t t ⎰.1. Separation Variable()()()()dy M x N y dy M x dx dx N y =⇒=⎰⎰ 2. Logistic Equation (1)(),lim ()1kt t dP P K kP P t P t K dt K e-→∞=-⇒==+ ,where is the environmental capacity. 3. Slope Field4. Euler’s Method : 11n n n n x x x dyy y x dx ++=+∆⎧⎪⎨=+⋅∆⎪⎩n a ++,1n a∞=∑Test for ConvergenceAssume that the following limit exists: 1≥..③ Comparison TestAssume that there exists 0M >such that 0n n a b ≤≤for n M ≥. If1nn b∞=∑ converges, then If1nn a∞=∑ also converges. If1nn a∞=∑ diverges, then1nn b∞=∑ alsodiverges.Limit Comparison Test Let {}n a and {}n b be positive sequences. Assume limnn na Lb →∞=a) If 0L >,then1nn a∞=∑ converges if and only if1nn b∞=∑ converges.b) If L =∞, and1nn a∞=∑ converges, then1nn b∞=∑ converges.c) If 0L =, and1nn b∞=∑ converges, then1nn a∞=∑ converges.3. Four Series① The geometric seriesnar∞converges if ||1r <and diverges otherwise.0, lim n n a a →∞>>>>4. ()(012a a x c a x +-+-()n∞∑is either anonnegative number ( )or infinity( ).If i s finite, converges absolutely whenx c R -< and diverges when x c R ->. If R =∞, ()F x converges absolutely for all x .(ii )Term-by-term Differentiation and IntegrationPossible convergence at the endpointsAssume that ()0()nn n F x a x c ∞==-∑ has radius of convergence 0R > . Then is differentiable on(, )c R c R -+[or for all x if R =∞]. Furthermore, we can integrate and differentiate term by term.For (, )x c R c R ∈-+,()()110'() () (A any constant)1n n n n n n a F x na x c F x dx x c A n ∞∞-+===-=-++∑∑⎰;These series have the same radius of convergence R .()(!n f a n ++()(0)!n f n ++and x ).Interval of convergence all real numbers初等函数基本知识点小结一、指数函数(一)指数与指数幂的运算1.∈N◆2ma n◆3(112(一)对数1.对数的概念:一般地,如果N a x =)1,0(≠>a a ,那么数x 叫做以.a 为底..N 的对数,记作:N x a log =(a — 底数,N — 真数,N a log — 对数式)说明:注意底数的限制0>a ,且1≠a 两个重要对数:○1 常用对数:以10为底的对数N lg ; ○2 自然对数:以无理数 71828.2=e 为底的对数N ln . 指数式与对数式的互化幂值 真数如果a ○1 ○2 ○3 abln ln(1)1,+∞). ○2 21、幂函数定义:一般地,形如αx y =)(R a ∈的函数称为幂函数,其中α为常数.2、幂函数性质归纳.(1)所有的幂函数在(0,+∞)都有定义并且图象都过点(1,1);(2)0>α时,幂函数的图象通过原点,并且在区间),0[+∞上是增函数.特别地,当1>α时,幂函数的图象下凸;当10<<α时,幂函数的图象上凸;(3)0<α时,幂函数的图象在区间),0(+∞上是减函数.在第一象限内,当x 从右边趋向原点时,图象在y 轴右方无限地逼近y 轴正半轴,当x 趋于∞+时,图象在x 轴上方无限地逼近x 轴正半轴.三角函数基本知识点小结sin1. 2. 3.sin(α±β(tan a a sin2αa 2tan =Trigonometric Function and Inverse Trigonometric Function。
ap分子微积分bc学

AP分子微积分BC学1. 什么是AP分子微积分BC学?AP分子微积分BC学是一门高级的微积分课程,旨在为学生提供更深入的微积分学习和应用的机会。
它是AP微积分AB学的进阶课程,涵盖了更多的内容和更高的难度。
2. AP分子微积分BC学的课程内容AP分子微积分BC学的课程内容主要包括以下几个方面:2.1 微分学微分学是微积分的基础,它研究函数的变化率和极限概念。
在AP分子微积分BC学中,学生将进一步学习导数的计算和应用,包括高阶导数、隐函数求导、参数方程求导等。
此外,还将学习微分中值定理、泰勒展开等重要概念和定理。
2.2 积分学积分学是微积分的另一个重要分支,它研究函数的面积、曲线长度、体积等问题。
在AP分子微积分BC学中,学生将学习不定积分和定积分的计算和应用,包括分部积分、换元积分、定积分的应用等。
此外,还将学习积分学中的重要定理,如牛顿-莱布尼兹公式等。
2.3 微分方程微分方程是微积分的应用领域之一,它研究函数与其导数之间的关系。
在AP分子微积分BC学中,学生将学习一阶和二阶微分方程的解法,包括分离变量法、齐次方程法、常系数线性方程法等。
此外,还将学习微分方程的应用,如生物学中的人口模型、物理学中的运动模型等。
2.4 级数和序列级数和序列是微积分中的重要概念,它们用于研究无穷和。
在AP分子微积分BC学中,学生将学习级数和序列的收敛性和发散性,以及级数和序列的计算和应用。
此外,还将学习级数和序列的重要定理,如比较判别法、积分判别法等。
2.5 三维空间几何三维空间几何是微积分的应用领域之一,它研究三维空间中的点、直线、平面等几何概念。
在AP分子微积分BC学中,学生将学习三维空间几何的基本概念和计算方法,包括点到直线的距离、平面方程等。
此外,还将学习三维空间几何在微积分中的应用,如曲线长度、曲面面积等。
3. AP分子微积分BC学的考试要求AP分子微积分BC学的考试要求包括两个部分:选择题和解答题。
选择题部分主要考察学生对微积分概念和定理的理解和运用能力,要求学生能够正确计算导数、积分、微分方程等,并能够分析和解释函数的性质和图像。
AP微积分BC 5分指南】AP Calculus BC

AP微积分BC 5分指南】APCalculus BCAP Calculus BCAP 微积分BC学科介绍微积分BC考试比微积分AB考试大约多出30%的考点,一般而言,如果是国内优质高中或者重点高中背景的考生,比较建议一步到位考微积分BC。
如果是国际高中或者美国高中,比较建议顺着学校的安排,一般而言,此类学校是建议考生先考微积分AB再考微积分BC,注意AP考试一年一次。
学科目的帮助学生掌握微积分的知识,培养学生专业的数学学科思维方式,熟悉并养成学科研究范式,在学习过程中体味数学的乐趣,掌握数学思维,顺利的通过AP微积分ab考试.学科内容Limit and Continuity 极限和连续极限的定义和左右极限极限的运算法则和有理函数求极限两个重要的极限极限的应用-求渐近线连续的定义三类不连续点(移点、跳点和无穷点)最值定理、介值定理和零值定理Part2:Derivative 导数导数的定义、几何意义和单侧导数极限、连续和可导的关系导数的求导法则(共21个)复合函数求导高阶导数隐函数求导数和高阶导数反函数求导数参数函数求导数和极坐标求导数Part3. Application of Derivative 导数的应用微分中值定理(D-MVT)几何应用-切线和法线和相对变化率物理应用-求速度和加速度(一维和二维运动)求极值、最值,函数的增减性和凹凸性洛比达法则求极限微分和线性估计,四种估计求近似值欧拉法则求近似值Part4. Indefinite Integral 不定积分不定积分和导数的关系不定积分的公式(18个)换元法求不定积分部积分法求不定积分待定系数法求不定积分Definite Integral 定积分Riemann Sum(左、右、中和梯形)和定积分的定义和几何意义牛顿-莱布尼茨公式和定积分的性质Accumulation function求导数反常函数求积分Part6:Application of Integral 定积分的应用积分中值定理(I-MVT)定积分求面积、极坐标求面定积分求体积,横截面体积求弧长定积分的物理应用Differential Equation微分方程可分离变量的微分方程和逻辑斯特微分方程斜率场Part7: Infinite Series 无穷级数无穷级数的定义和数列的级三个审敛法-比值、积分、比较审敛法四种级数-调和级数、几何级数、P级数和交错级数函数的级数-幂级数(收敛半径)、泰勒级数和麦克劳林级数级数的运算和拉格朗日余项、拉格朗日误差Mock exam 模拟考试Past paper review 历年试卷回顾考核形式考试有45到多选题和6到简答题两个部分,每个部分又根据能否使用计算器而分两个Part,不允许跨区答题。
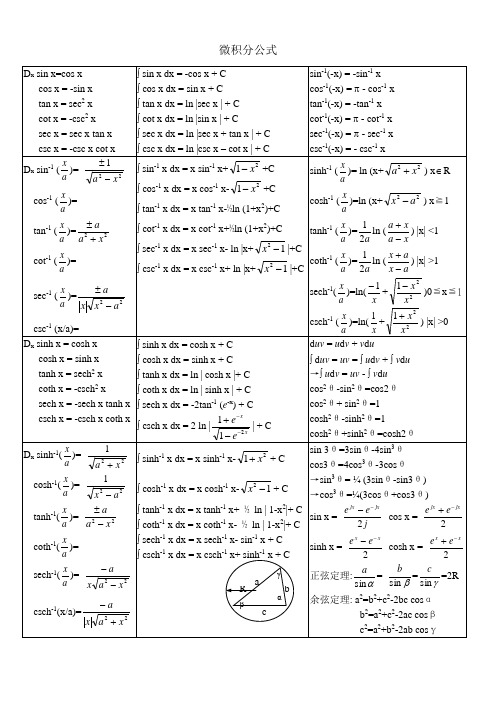
微积分公式
p np
kq p
pq npq
kq p2
q+pet (q+ pet)n
pk (1 − qe t ) k
npi
1 p k n N
npi(1-pi)
q p2 N −n k n N −1 N
=
∫
∞
0
x m −1 dx (1 + x ) m+ n
希腊字母 (Greek Alphabets) 大写 Α Β Γ Δ Ε Ζ Η Θ 小写
α β γ δ ε ζ η θ
读音 alpha beta gamma delta epsilon zeta eta theta
大写 Ι Κ Λ Μ Ν Ξ Ο Π
小写
ι κ λ μ ν ξ ο π
读音 iota kappa lambda mu nu xi omicron pi
大写 Ρ Σ Τ Υ Φ Χ Ψ Ω
小写
ρ σ, ς sigma tau τ upsilon υ phi φ khi χ psi ψ omega ω
读音 rho
倒数关系: sinθcscθ=1; tanθcotθ=1; cosθsecθ=1 商数关系: tanθ=
机率函数 f(x)
1 n 1 b−a
期望值 E(x)
1 (n+1) 2 1 (a+b) 2
变异数 V(x)
1 2 (n +1) 12 1 (b-a)2 12
动差母函数 m(t)
1 e t (1 − e nt ) n 1 − et
AP微积分考试最全介绍

AP微积分考试最全介绍AP微积分考试最全介绍三立为大家整理了关于AP微积分考试史上最全的介绍,供大家参考,希望对大家有所帮助!一.考试介绍AP微积分是为了致力读美国本科的高中生所设立的考试,在AP 微积分中取得好成绩的学生可以获得大学期间微积分课程的免修课程,而绝大多数理工科以及经济学专业在大学期间都需要修微积分的课,所以AP微积分对绝大多数专业都是非常必要的。
AP微积分分为AB和BC,其中BC的难度要高于AB,现在绝大多数美国的学校更愿意接受AP微积分BC的成绩。
AP微积分分为选择题和简答题,两部分都进一步分为A部分和B 部分。
选择题中,A 部分28题,时间为55分钟,在这部分中,学生不允许使用计算器;B部分17题,时间为50分钟,学生可以使用计算器。
简答题中,A部分两道题,时间为30分钟,学生可以使用计算器;B部分四道题,时间为60分钟,学生不可以使用计算器。
AP微积分总分为108分,但这只是“未经加工的分数(raw score)”,也就是卷面分数,而真正重要的是最后的实际分数。
实际分数分为1-5五个档次,只有5分和4分才有可能被美国高校所接受。
在AP微积分考试中,只要获得65分左右的“未经加工的分数”,就可以获得最高档次的5分,所以说AP微积分考试是一个“性价比”比较高的考试。
二.考试内容1. AP微积分BC考试内容a. 极限和连续b. 导数及其应用(包括参数方程和极坐标)c. 不定积分d. 定积分及其应用(包括极坐标)e. 微分方程解法及应用f. 级数及其应用2. AP微积分AB考试内容在AP微积分BC的内容中,去掉有关分部积分法、级数、Logistic增长、参数方程和极坐标以及物理向量方面的内容,其他内容基本保持一致。
三.备考策略1. 必备教材和材料Barron AP微积分,世界图书出版社。
AP微积分简答题历年真题(College Board官网下载)。
2. 时间规划(1)AP微积分AB建议从3月开始备考,直到5月考试结束。
ap微积分bc课程知识点

ap微积分bc课程知识点AP微积分BC课程知识点AP微积分BC课程是高中数学的一门重要课程,它主要涉及微积分和计算机科学方面的知识,是一门比较难度较大的课程。
本文将从以下几个方面详细介绍AP微积分BC课程的知识点。
一、微积分基础1.导数导数是微积分中最基础的概念之一,它表示函数在某个点上的变化率。
在AP微积分BC课程中,我们需要掌握导数的定义、求导法则、高阶导数等内容。
2.极限极限是微积分中另一个重要概念,它表示函数在某个点上趋近于某个值的过程。
在AP微积分BC课程中,我们需要掌握极限的定义、性质、计算方法等内容。
3.泰勒级数泰勒级数是一种用多项式逼近函数的方法,在AP微积分BC课程中也有所涉及。
我们需要掌握泰勒级数的定义、求法、误差估计等内容。
二、微积分应用1.曲线拟合曲线拟合是指用一个函数来逼近离散数据点的过程,在AP微积分BC 课程中也有所涉及。
我们需要掌握曲线拟合的原理、方法、误差估计等内容。
2.微积分基本定理微积分基本定理是微积分中最重要的定理之一,它将导数和积分联系起来。
在AP微积分BC课程中,我们需要掌握微积分基本定理的两个部分以及它们的应用。
3.微积分应用微积分在各个领域都有广泛的应用,如物理学、经济学、工程学等。
在AP微积分BC课程中,我们需要掌握微积分在不同领域中的应用,如求面积、体积、速度、加速度等。
三、计算机科学1.算法设计与复杂度算法设计与复杂度是计算机科学中最重要的概念之一,它涉及到算法正确性和效率问题。
在AP微积分BC课程中,我们需要掌握算法设计与复杂度的基本概念以及常见算法的时间复杂度。
2.数据结构数据结构是计算机科学中另一个重要概念,它涉及到数据在计算机内部存储和处理的方式。
在AP微积分BC课程中,我们需要掌握常见数据结构的定义、实现和应用。
3.离散数学离散数学是计算机科学中的一门基础课程,它涉及到集合论、图论、逻辑等内容。
在AP微积分BC课程中,我们需要掌握离散数学的基本概念以及应用。
ap微积分公式大全

ap微积分公式大全AP微积分是高中阶段最常见的一门数学课程之一,涉及到许多基本的微积分概念和公式。
掌握这些公式对于理解微积分的原理和应用非常重要。
下面是一份AP微积分公式的大全,旨在帮助学生们更好地应对这门课程。
1. 极限:- 无穷限极限:lim(x → ∞) f(x) = L- 无穷小限极限:lim(x → 0) f(x) = L- x → 0 时,lim(x → 0) f(x) = L2. 导数:- 基本导数法则:(d/dx)(c) = 0,(d/dx)(x^n) = n*x^(n-1),(d/dx)(e^x) = e^x,(d/dx)(sin(x)) = cos(x),(d/dx)(cos(x)) = -sin(x)- 乘法法则:(d/dx)(f(x)*g(x)) = f"(x)*g(x) + f(x)*g"(x) - 链式法则:(d/dx)(f(g(x))) = f"(g(x))*g"(x)3. 积分:- 基本积分法则:∫(c) dx = cx,∫(x^n) dx =(x^(n+1))/(n+1) + C,∫(e^x) dx = e^x + C,∫(sin(x)) dx = -cos(x) + C,∫(cos(x)) dx = sin(x) + C- 定积分:∫[a, b] f(x) dx4. 微分方程:- 一阶常微分方程:dy/dx + P(x)y = Q(x)- 分离变量法:dy/y = P(x)dx5. 泰勒级数:- 泰勒级数展开:f(x) = f(a) + f"(a)(x-a) +(1/2!)f""(a)(x-a)^2 + (1/3!)f"""(a)(x-a)^3 + ...6. 极值和最值:- 极值:f"(x) = 0,f""(x) > 0 (极小值) 或 f""(x) < 0 (极大值)- 最值:在定义域内找到函数的最大值和最小值以上列举的只是AP微积分中的一部分基本公式,对于每个公式的具体应用和推导过程,还需要进一步深入学习和理解。
AP微积分BC考试知识点梳理

AP微积分BC考试知识点梳理大家在最后冲刺地时候应该主要以下几点.1,梳理公式〔导数表,积分表,特殊角的三角函数值,三角公式〔主要是二倍角公式〕〕.2,理解主要概念:导数〔瞬时变化率〕,criticle驻点,inflection拐点,极值与最值,水平渐近线和垂直渐近线.3. 掌握几种方法.第一求极限的方法1. 分式型〔直接代入,约分后代> ;2.导数的极限形式;3.不定型与洛必达法则.第二求导数的方法1. 乘法、除法法则2. 复合函数的链式法则3. 隐函数求导4. 参数方程求导第三求积分的方法1. 第一换元法2. 分部积分与表格法3. 部分分式〔未掌握可以忽略了〕第四函数值的近似1. 切线近似2. 欧拉方法第五积分的近似1. 黎曼和2. 矩形近似〔左右中点〕3. 梯形近似4. 无穷级数近似.第六体积1. 截面是正方形,垂直于横轴2. 旋转截面是圆或环.第七微分方程分离变量第八速度加速度1.区分速度与速率2.区分路程与位移第九无穷级数1. 泰勒展开的通式2. 逐项积分3. 逐项求导4. 近似级数5. 比例法求收敛半径6. 误差分析〔没掌握就放弃〕4,几个主要定理1. 拉格朗日中值定理2. 微积分基本定理一3. 微积分基本定理二只要掌握了这些基本的主干知识点,就可以轻松地得5分了,其他的知识可以忽略不计了〔如广义积分,极坐标,收敛性的判定等〕.以下总结各种知识点,仅供查漏补缺....1.梳理公式A.微分B.积分除这些基本公式以外还有csc, sec,tan, arcsin, arccos, cot神马的各种公式,考得不多但目标5分的各位可以在考前翻出来熟悉一下.C.特殊角的三角函数值D. 三角公式〔主要是二倍角公式〕2. f<x>图像里重要概念:if f<x> is continuous anddifferentiable.a. 导数〔瞬时变化率〕f<x> f'<x> f''<x>s v ab. criticle points 驻点f'<x>=0 stationary pointsc. inflection points 拐点f ''<x>=0 f '<x>≠0d. local<relative> max&min 最大值最小值max f'<x>=0 f ''<x><0min f'<x>=0 f ''<x>>0e. Sign test 符号测试法left rightmax + -min - +horizentalinflection - /+ -/+f. Features of fraph 图像特征f'<x>>0 increasingf'<x><0 dcreasingf ''<x>>0 concave upf''<x>>0 concave down3. 需要掌握的一些重要方法一. 求极限的方法1. 分式型〔直接代入,约分后代> ;2. 导数的极限形式;3. 不定型与洛必达法则<分子分母同时微分>.二. 求导数的方法1. 乘法、除法法则2. 复合函数的链式法则3. 隐函数求导4. 参数方程求导三. 求积分的方法1. 第一换元法〔假设〕2.分部积分与表格法*积分式中如有X与ln则设为u四. 函数值的近似1.切线近似2. Euler's Method <欧拉方法>Yn = Yn-1 + Y 'n-1 * h五. 积分的近似1. Riemann Sum <黎曼求和>Subintervals: Rantangles〔左右中点〕2. Trapezium Rule <梯形法则>3. 无穷级数近似六. 体积1. Cross Section <由横截面得到,垂直X轴或Y轴>2. Solid of Revolution <旋转, 截面是圆或环>七. 微分方程1. 分离变量将dy与y放到等号一边 dx与x另一边然后等号两边同时积分八. 速度加速度1.区分速度<velocity>与速率<speed>2.区分路程<distance>与位移<displacement>九. 无穷级数1.Taylor Series展开通式2. 近似级数3. 比例法求收敛半径| an+1 / an | < 1converge n→Infinite4. Lagrange error bound <拉格朗日误差分析〕十. Area in Polar Form <极坐标方程求面积>x=rcosαy=rsinαA=1/2 ∫r²dα <由α1积到α2>十一. Motion along a curve <曲线运动,弧长>另外几个需要熟悉的特殊泰勒展开式:。
AP微积分-AP Calculus 公式大全-259

AP Calculus BC1. Important limits()001111011sin sin lim1, lim 1lim 1lim 10, ()lim lim , x x xt x t m m m m m m n n x ax ax bx be t e x m n P x a x a x a x a a m n b x b →→→∞→−−−==⎛⎫+=⇔+= ⎪⎝⎭<++++===+++⎧⎪⎪⎨x x 2sec )'(tan = x 2csc (cot)'−= x x x tan sec )'(sec = x x x cot csc )'(csc −=(6) 211)'(arcsin x x −=211)'(arccos xx −−=211)'(arctan x x +=211)'cot (x x ar +−= 11)'sec (2−=x x x arc 11)'csc (2−−=x x x arc2. Rules(1)If f (x ),g (x ) are differential ,a. )()())()((x g x f x g x f '±'='±;b. )()()()())()((x g x f x g x f x g x f '+'=',especially ,)())((x f C x Cf '='(C is a constant );c. )0)(( ,)()()()()())()((2≠'−'='x g x g x g x f x g x f x g x f ,especially ,21()()()()g x g x g x ''=−。
(2) Chain Rule )]([x g f y =⇒dxdudu dy dx dy ⋅= (3) Implicit Differentiation ()(()'r t f =3. Applications of Derivative Mean Value Theorem) is differentiable Tangent and normalFirst Derivative Test'f changes from − to +,local min 'f changes from + to −,local maxSecond Derivative Test ''()0f a >,local min ''()0f a <,local max(4) Absolute max/min: compare function value of critical point and endpoints.Steps: 1. find 'f ;2.solve '0f =;3. compare value at critical point and endpoints.1. Indefinite Integral:'()()Definition f x dx f x C =+⎰ Method ()()df x f x C =+⎰2. Methods for Indefinite Integral 不定积分方法 ① Formulas :dx x C =+⎰(integrand 为1), kdx kx C =+⎰, 11n nx x dx C n +=++⎰ln x xa a dx C a=+⎰ x xe dx e C =+⎰1sin 2 4x C ++②()f u du3. Find the antiderivative of ()f u .③ Partial fraction (拆分)ln ||ln ||()()cx d A Bdx dx dx A x a B x b C x a x b x a x b +=+=−+−+−−−−⎰⎰⎰(通分求A,B)④ Integration by partsudv uv vdu =−⎰⎰,(or ''uv dx uv vu dx =−⎰⎰), Tabular Integration , (story about ln x , sin x , and xe )3. Improper Integral 反常积分(两种形式,无穷积分,瑕积分) ① Integral on infinite interval()lim (),()lim (),()()()lim ()lim ().ba ab bbaa c cbcaca b f x dx f x dx f x dx f x dx f x dx f x dx f x dx f x dx f x dx ∞→∞−∞→−∞∞∞−∞−∞→−∞→∞===+=+⎰⎰⎰⎰⎰⎰⎰⎰⎰② Integrand with infinite discontinuities()lim (),bca ac bbbf x dx f x dx −→=⎰⎰4.5. )'()x β⋅6. ①(,)c a b ∈. ②low upper function er function a ⎣⎦L Right functio ef func on n ti )cy dy ⎤⎥⎣⎦③ V olume :with known cross section()baA x dx ⎰, ()A x is the area of the cross section.Revolution :2Inner 2Out rad er radiu ius s baR r dx π⎡⎤−⎣⎦⎰,Shell Method :2baxydx π⎰(旋转轴为y-axis ) or 2baxydy π⎰(旋转轴为x-axis )④ Length21x x ⎰or 21y y ⎰or 21t t ⎰.1. Separation Variable()()()()dy M x N y dy M x dx dx N y =⇒=⎰⎰ 2. Logistic Equation (1)(),lim ()1kt t dP P K kP P t P t K dt K e−→∞=−⇒==+ ,where is the environmental capacity. 3. Slope Field4. Euler’s Method : 11n n n n x x x dyy y x dx ++=+∆⎧⎪⎨=+⋅∆⎪⎩n a ++,1n a∞=∑Test for ConvergenceAssume that the following limit exists:1≥..③ Comparison TestAssume that there exists 0M >such that 0n n a b ≤≤for n M ≥. If1nn b∞=∑ converges, then If1nn a∞=∑ also converges. If1nn a∞=∑ diverges, then1nn b∞=∑ alsodiverges.Limit Comparison Test Let {}n a and {}n b be positive sequences. Assume limnn na Lb →∞=a) If 0L >,then1nn a∞=∑ converges if and only if1nn b∞=∑ converges.b) If L =∞, and1nn a∞=∑ converges, then1nn b∞=∑ converges.c) If 0L =, and1nn b∞=∑ converges, then1nn a∞=∑ converges.3. Four Series① The geometric seriesnar∞converges if ||1r <and diverges otherwise.0, lim n n a a →∞>>>>4. ()(012a a x c a x +−+−∞is either anonnegative number (0R ≥ )or infinity(R =∞ ).If R i s finite, ()F x converges absolutely whenx c R −< and diverges when x c R −>. If R =∞, ()F x converges absolutely for all x .(ii )Term-by-term Differentiation and IntegrationPossible convergence at the endpointsAssume that ()0()nn n F x a x c ∞==−∑ has radius of convergence 0R > . Then is differentiable on(, )c R c R −+[or for all x if R =∞]. Furthermore, we can integrate and differentiate term by term.For (, )x c R c R ∈−+,()()110'() () (A any constant)1n n n n n n a F x na x c F x dx x c A n ∞∞−+===−=−++∑∑⎰;These series have the same radius of convergence R . ()(!n f a n ++()(0)!n f n ++and x ).Interval of convergence all real numbers初等函数基本知识点小结一、指数函数1.∈N◆2ma n◆3(112(一)对数1.对数的概念:一般地,如果N a x =)1,0(≠>a a ,那么数x 叫做以.a 为底..N 的对数,记作:N x a log =(a — 底数,N — 真数,N a log — 对数式) 说明:注意底数的限制0>a ,且1≠a两个重要对数:○1 常用对数:以10为底的对数N lg ; ○2 自然对数:以无理数 71828.2=e 为底的对数N ln . ◆ 指数式与对数式的互化幂值 真数如果a ○1 ○2 ○3 abln ln(1)1,+∞). ○2 21、幂函数定义:一般地,形如αx y =)(R a ∈的函数称为幂函数,其中α为常数. 2、幂函数性质归纳.(1)所有的幂函数在(0,+∞)都有定义并且图象都过点(1,1);(2)0>α时,幂函数的图象通过原点,并且在区间),0[+∞上是增函数.特别地,当1>α时,幂函数的图象下凸;当10<<α时,幂函数的图象上凸;(3)0<α时,幂函数的图象在区间),0(+∞上是减函数.在第一象限内,当x 从右边趋向原点时,图象在y 轴右方无限地逼近y 轴正半轴,当x 趋于∞+时,图象在x 轴上方无限地逼近x 轴正半轴.三角函数基本知识点小结sin1. 2. 3.sin(α±β(tan a a sin 2αa 2tan =Trigonometric Function and Inverse Trigonometric Function。
ap微积分公式大全

ap微积分公式大全1.函数相关公式:-函数定义域:若函数f(x)在x=a处有定义,则a属于f(x)的定义域。
-函数值:当x=a时,函数f(x)的值记作f(a)。
-函数奇偶性:-若对于任意x,f(-x)=-f(x),则f(x)为奇函数。
-若对于任意x,f(-x)=f(x),则f(x)为偶函数。
-两个函数的和差乘除:-(f+g)(x)=f(x)+g(x)-(f-g)(x)=f(x)-g(x)- (fg)(x) = f(x) * g(x)-(f/g)(x)=f(x)/g(x),其中g(x)≠0。
-复合函数:-(f∘g)(x)=f(g(x))2.极限相关公式:-极限定义:- 若对于任意ε>0,存在δ>0,使得当0 < ,x - a,< δ时,有,f(x)-L,< ε,则称函数f(x)在x=a处的极限为L,记作lim┬(x→a)〖f(x) = L〗。
-基本极限:- lim┬(x→∞)(1/x) = 0- lim┬(x→0)(sinx/x) = 1- lim┬(x→0)(eˣ-1)/x = 1- lim┬(x→0)(ln(1+x)/x) = 1-极限的四则运算:- lim┬(x→a)(f(x) ± g(x)) = lim┬(x→a)(f(x)) ±lim┬(x→a)(g(x))- lim┬(x→a)(cf(x)) = c * lim┬(x→a)(f(x)),其中c为常数。
- lim┬(x→a)(f(x)g(x)) = lim┬(x→a)(f(x)) *lim┬(x→a)(g(x))- lim┬(x→a)(f(x)/g(x)) = lim┬(x→a)(f(x)) /lim┬(x→a)(g(x)),其中lim┬(x→a)(g(x))≠0。
3.导数相关公式:-导数定义:- 若极限lim┬(h→0)〖(f(x+h) - f(x))/h 〗存在,则称这个极限为函数f(x)在x点的导数,记作f'(x)。
ap微积分ab和bc中文讲义

AP微积分AB和BC是大学预修课程,主要涉及微积分的基础知识。
以下是它们的中文讲义:一、AP微积分AB1. 极限与连续极限是研究函数在某一点附近的行为,分为数列极限和函数极限。
连续是指函数在某一点的极限存在且等于该点的函数值。
2. 导数导数表示函数在某一点的切线斜率,反映了函数在该点的变化率。
导数的计算方法有导数的定义、导数的几何意义和导数的物理意义。
3. 微分微分是导数的另一种表现形式,表示函数在某一点的局部变化量。
微分的计算方法有微分的定义、微分的几何意义和微分的物理意义。
4. 不定积分不定积分是求原函数的过程,分为基本不定积分和复合不定积分。
不定积分的计算方法有换元法、分部积分法和有理函数积分法。
5. 定积分定积分是求曲线下面积的过程,分为不定积分和定积分。
定积分的计算方法有牛顿-莱布尼茨公式、数值积分法和几何应用。
二、AP微积分BC1. 多元函数微分学多元函数是指有两个或两个以上自变量的函数。
多元函数的极限、连续性、偏导数、全微分等概念与单变量函数类似,但需要考虑多个自变量之间的关系。
2. 多元函数积分学多元函数的积分是指求多元函数在某一区域内的平均值或总和。
多元函数的重积分、多重积分和曲线积分等概念与单变量函数类似,但需要考虑多个自变量之间的关系。
3. 微分方程微分方程是描述变量之间关系的方程,分为常微分方程和偏微分方程。
常微分方程的解法有分离变量法、一阶线性微分方程、二阶常系数齐次线性微分方程和二阶常系数非齐次线性微分方程等。
偏微分方程的解法有分离变量法、格林公式、高斯公式等。
apcalculusbc知识点

apcalculusbc知识点AP Calculus BC是一个高级的高中数学课程,相比AP Calculus AB 更加细致深入。
本篇文章将介绍AP Calculus BC的主要知识点。
1.极限和连续性:-无穷极限和端点极限-洛必达法则-弧长和速度-曲率和切线-渐近线2.微分学:-导数定义和基本性质-高阶导数-非常量、参数方程和隐函数的导数-高阶导数的应用,如极值点、拐点和凸凹性3.积分学:-不定积分和定积分-定积分的性质和应用-分部积分法-微元法和参数方程的积分-应用积分,如平均值、体积和弧长4.微分方程:-各种类型的微分方程,如一阶线性方程、分离变量方程和二阶线性方程-特解和通解-平衡解和稳定解-初值问题的解法5.数列和级数:-数列的性质和极限-级数的性质和部分和-调和级数和几何级数-收敛和发散的判断-收敛级数的运算6.极坐标和向量:-极坐标的定义和性质-极坐标下的导数和积分-向量的运算和性质-向量场和向量值函数7.空间几何和曲线:-三维空间中的曲线和曲面-空间曲线的参数方程和切向量-曲线的弧长和曲率-空间曲面的切平面和法向量8. 一元函数的Taylor级数:- Taylor级数的定义和性质- Taylor级数的收敛区间和收敛速度- Taylor级数在数学和物理中的应用9.极值和最优化问题:-极值的定义和判定条件-最优化问题的建模和求解-约束条件下的最优化问题-拉格朗日乘数法10.空间曲面的积分:-曲面积分的定义和计算-参数化曲面和曲面元素-曲面积分的应用,如质心和质量11.多元函数的积分学:-二重积分的定义和计算-极坐标下的二重积分-三重积分的定义和计算-柱坐标和球坐标下的三重积分12.向量值函数和曲线积分:-向量值函数的导数和积分-向量值函数的积分曲线和曲线元素-曲线积分的计算和应用- 曲线积分的Green定理这些是AP Calculus BC的主要知识点,它们涵盖了微积分的核心概念和应用。
{无敌必备}AP微积分公式大全

f ( x) dx lim f ( x)dx
a a
b
f ( x) dx
f ( x )dx
b
f ( x )dx
(2) Integrand with Infinite discontinuities
b a b
f ( x) dx lim f ( x )dx
c a c
a b
f ( x) dx lim f ( x) dx
cb c a
c
a
f ( x) dx f ( x)dx f ( x )dx
a c
b
4. 定积分定义和运算法则
(1) Riemann sum(left , right , mid , trapezoidal ) (2) definition : f ( x )dx lim Ai lim f ( xi )x
a n n b
(3) properties of Integral :
3
Made by Yong Luo @XDF ---------------------------------------------------------------------------------------------------------
1. 不定积分定义式 definition f '( x) dx f ( x) C 2. 求不定积分的四种方法
method df ( x) f ( x) C;
(1) Formulas : x n dx
x n 1 1 u a C C , a u du n 1 ln a
Chapter1. Function and Limit
AP微积分BC公式大全

dy dy dt d 2 y d (dy dx ) dt = , = dx dx dt dx 2 dx dt ���� dy f '(θ ) sin θ + rcosθ polar = , vector (r (t )) ' =< f '(t ), g '(t ) > dx f '(θ ) cos θ − r sin θ parametric
AP 微积分公式大全
AP 微积分考试为闭卷考试,考试时也不给任何数学公式。因此,熟练掌握考试大 纲要求的微积分公式十分必要。为了帮助考生更快的掌握 AP 微积分的相关公式, 本文总结了 AP 微积分考试当中常用的重要公式,供大家参考学习。
Chapter1. Function and Limit
1. 5 种基本初等函数图像性质 Power y = x µ ; Exponential y = a x ; Logarithmic : y = log a x
∞
(1)∑ an = a1 + a2 + a3 + ..... + an + .....;
n =1 n
(2) partial sum : Sn = ∑ an = a1 + a2 + a3 + ..... + an ;
n =1
∞
(3) If lim S n exits, then ∑ an converges;
(cos x) ' = − sin x,
(cot x) ' = − csc 2 x, (sec x) ' = sec x tan x, (csc x) ' = − csc x cot x; 1 1 1 (arcsin x)′ = , (arccos x)′ = − , (arctan x)′ = , 2 2 2 1 + x 1− x 1− x 1 1 1 (arc cot x)′ = − , (arc sec x )′ = , (arc cot x )′ = − 2 1+ x | x | x2 −1 | x | x2 −1 u u ' v − uv ' (2)Operation : (u ± v) ' = u '± v ', (uv) ' = u ' v + uv ', ( ) ' = ; v v2 dy dy du (3)Chain rule y = f [ g ( x)] ⇒ = ⋅ dx du dx (4) Logarithmic differentiation dy 1 (5) Inverse function = dx dx dy
ap微积分公式表

ap微积分公式表以下是一些常见的AP微积分公式:1. 导数公式:- 常数函数导数:(c)' = 0- 幂函数导数:(x^n)' = nx^(n-1)- 指数函数导数:(e^x)' = e^x- 对数函数导数:(ln(x))' = 1/x- 三角函数导数:(sin(x))' = cos(x), (cos(x))' = -sin(x), (tan(x))' = sec^2(x)2. 基本积分公式:- 幂函数积分:∫x^n dx = (1/(n+1))x^(n+1) + C,其中C为常数- 指数函数积分:∫e^x dx = e^x + C- 对数函数积分:∫(1/x) dx = ln|x| + C- 三角函数积分:∫sin(x) dx = -cos(x) + C, ∫cos(x) dx = sin(x) + C, ∫sec^2(x) dx = tan(x) + C3. 特殊积分公式:- 分部积分法:∫u dv = uv - ∫v du- 替换法:∫f(g(x)) g'(x) dx = ∫f(u) du,其中u = g(x)- 定积分:∫[a, b] f(x) dx 表示函数f(x)在区间[a, b]上的面积或曲线下的面积4. 微分方程公式:- 一阶线性微分方程:dy/dx + P(x)y = Q(x),其中P(x)和Q(x)为已知函数- 二阶线性齐次微分方程:d^2y/dx^2 + p(x)dy/dx + q(x)y = 0,其中p(x)和q(x)为已知函数这只是一些常见的公式,AP微积分还涉及到更多的概念和技巧。
在学习过程中,建议参考教材或课程提供的公式表,并根据具体的题目和问题进行学习和应用。
高中ap微积分bc

高中AP微积分BC一、微积分的基础概念1. 导数和极限•导数的定义•导数的计算方法•导数的应用2. 不定积分•不定积分的定义•牛顿-莱布尼茨公式•不定积分的计算方法3. 定积分•定积分的定义•定积分的计算方法•定积分的应用二、微积分的进阶知识1. 级数与数列•数列的收敛与发散•级数的概念与性质•级数的收敛测试方法2. 傅里叶级数•傅里叶级数的定义•傅里叶级数的性质•傅里叶级数的应用3. 微分方程•微分方程的概念与分类•一阶线性微分方程的解法•二阶常系数齐次微分方程的解法三、微积分的进一步应用1. 多元函数与偏导数•多元函数的定义与性质•偏导数的概念与计算方法•多元函数的极值及最优化问题2. 多重积分•二重积分的定义与计算方法•三重积分的定义与计算方法•多重积分的应用3. 空间曲线与曲面积分•曲线积分的定义与计算方法•曲面积分的定义与计算方法•空间曲线与曲面积分的应用四、微积分的高级话题1. 偏微分方程•偏微分方程的概念与分类•线性偏微分方程的解法•热传导方程与波动方程2. 向量场与曲线积分•向量场的概念与性质•曲线积分的向量场定义•曲线积分的计算方法3. 格林公式与斯托克斯定理•格林公式的概念与应用•斯托克斯定理的概念与应用•格林公式和斯托克斯定理的关系以上是关于高中AP微积分BC的全面介绍,从基础概念开始,逐步深入地探讨了微积分的各个方面。
对于每个概念和计算方法,都给出了详细的解释和相关的应用。
希望这篇文章能够帮助你更好地理解和掌握微积分的知识。
ap微积分bc公式

ap微积分bc公式
AP微积分BC公式包括:
1.数学定义域和值域:只有当函数的定义域和值域都是实数时,
才能将其定义为一个函数。
2.自变量求导:它是研究函数随自变量变化导致函数变化的过程,用于将函数转化成另一函数,同时可以求出函数的变化速率和斜率。
3.极限:当函数在某一点处取得极限值时,可以确定这个函数在
这个点处的导数;
4.连续性:函数只有当它在每一点处可以取得极限时,才可以称
之为连续函数;
5.泰勒公式:它是一个展开函数的式子,用来简化函数的计算;
6.一阶方程:又称一元一次方程,它是指由一元一次函数和常数
相结合形成的方程,即只有一个未知量的方程;
7.注意:如果一个一元一次方程有两个解,则它为相等方程;
8.埃尔米特法则:它是用于解决多项式方程的一种常用的技术;
9.梯度和散度:梯度用来描述函数的变化速率,而散度表明函数
在某一点处的变化方向;
10.曲线积分:当函数被积分时,可以求出它在坐标轴上的图像,
从而求出函数在一段间隔内的实际面积;
11.反常积分:又称集合积分,它是将函数的反常情况放入积分范
围中,使用不同的方法来计算函数的积分;
12.向量计算:向量的运算和它的基本概念(向量大小、方向、模
和角)有关,它主要用于计算三维或二维空间内的向量;
13.矢量函数:它是将函数映射成向量,可以使用埃尔米特法则进行求解,可以用于解决曲线上的最小值、最大值等问题。
ap微积分bc考点

ap微积分bc考点AP微积分BC考点AP微积分BC是一门高级的数学课程,它涵盖了微积分和一些其他的数学概念。
这门课程是由美国大学理事会(College Board)开发的,旨在为高中生提供更深入的数学知识,为他们未来进入大学做好准备。
以下将详细介绍AP微积分BC考点。
I. 微积分基础知识1. 函数概念:函数的定义、图像、性质等。
2. 极限和连续性:极限的定义、计算方法、极限存在定理;连续函数的定义、连续函数的性质。
3. 导数和微分:导数和微分的定义、求导法则、高阶导数;应用题目如最值问题等。
4. 积分:不定积分和定积分概念及其计算方法(牛顿-莱布尼茨公式)、换元法、部分分式法等。
II. 微积分进阶知识1. 微积分基本定理:第一类和第二类基本定理。
2. 科技应用题目:如物理学中运动问题,经济学中边际收益问题等。
3. 微积分与几何关系:曲线的弧长、曲率、曲线的面积等。
III. 微积分高阶知识1. 多元函数微积分:多元函数的定义和性质,偏导数、全微分等。
2. 多重积分:二重积分和三重积分概念及其计算方法,如极坐标法、柱坐标法等。
3. 向量值函数微积分:向量值函数的定义和性质,向量值函数的导数和微分等。
IV. BC考试特点1. BC考试时间比AB考试时间长,涵盖了更多的内容。
2. BC考试中会出现更多的多选题和解答题目。
3. BC考试中会有一些高阶知识点,如多元函数微积分和向量值函数微积分。
V. 如何备考BC考试1. 熟悉微积分基础知识,并掌握其应用方法。
2. 着重学习微积分进阶知识,如科技应用题目和几何关系问题。
3. 深入学习高阶知识点,并进行练习。
可以参加模拟测试来检验自己的掌握情况。
4. 注意解答题目时要清晰明了地表达思路,并且注意细节问题。
VI. 总结AP微积分BC考试是一门高级的数学课程,涵盖了微积分和其他数学概念。
备考时,需要掌握微积分基础知识、着重学习微积分进阶知识和深入学习高阶知识点。
此外,在解答题目时要清晰明了地表达思路,并注意细节问题。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Derivatives
Basic Properties/Formulas/Rules d ( cf ( x ) ) = cf ¢ ( x ) , c is any constant. ( f ( x ) ± g ( x ) )¢ = f ¢ ( x ) ± g ¢ ( x ) dx d n d x ) = nx n-1 , n is any number. ( c ) = 0 , c is any constant. ( dx dx æ f ö¢ f ¢ g - f g ¢ – (Quotient Rule) ( f g )¢ = f ¢ g + f g ¢ – (Product Rule) ç ÷ = g2 ègø d f ( g ( x ) ) = f ¢ ( g ( x ) ) g ¢ ( x ) (Chain Rule) dx g¢ ( x) d d g ( x) g x ln g ( x ) ) = e = g¢( x) e ( ) ( dx g ( x) dx
c b c
f ( x ) dx = ò f ( x ) dx + ò f ( x ) dx
a b
ò a c dx = c ( b - a )
f ( x ) dx ³ ò g ( x ) dx
a b
b
If f ( x ) ³ 0 on a £ x £ b then
ò a f ( x ) dx ³ 0 òa
d ( tan x ) = sec2 x dx d ( cot x ) = - csc2 x dx d 1 tan -1 x ) = ( dx 1 + x2 d 1 cot -1 x ) = ( dx 1 + x2
Exponential/Logarithm Functions d x d x a ) = a x ln ( a ) e ) = ex ( ( dx dx d 1 d 1 ln ( x ) ) = , x > 0 ln x ) = , x ¹ 0 ( ( dx x dx x Hyperbolic Trig Functions d ( sinh x ) = cosh x dx d ( sech x ) = - sech x tanh x dx d ( cosh x ) = sinh x dx d ( csch x ) = - csch x coth x dx
d 1 log a ( x ) ) = , x>0 ( dx x ln a d ( tanh x ) = sech 2 x dx d ( coth x ) = - csch 2 x dx
Visit for a complete set of Calculus I & II notes.
2 2 3
ò csc u du = lponential/Logarithm Functions
ò csc
u
3
u du =
1 ( - csc u cot u + ln csc u - cot u ) + c 2
òe
u
du = e + c
u
au ò a du = ln a + c
(
)
( )
Common Derivatives Polynomials d d (c) = 0 ( x) = 1 dx dx Trig Functions d ( sin x ) = cos x dx d ( sec x ) = sec x tan x dx Inverse Trig Functions d 1 sin -1 x ) = ( dx 1 - x2 d 1 sec -1 x ) = ( dx x x2 - 1
ò sin
-1
u du = u sin -1 u + 1 - u 2 + c 1 u du = u tan -1 u - ln (1 + u 2 ) + c 2 u du = u cos -1 u - 1 - u 2 + c
ò tan
-1
ò cos
-1
ò sech tanh u du = - sech u + c ò tanh u du = ln ( cosh u ) + c
© 2005 Paul Dawkins
Common Derivatives and Integrals
Integrals
Basic Properties/Formulas/Rules ò cf ( x ) dx = c ò f ( x ) dx , c is a constant.
b a b
ò f ( x ) ± g ( x ) dx = ò f ( x ) dx ± ò g ( x ) dx b b ò a f ( x ) dx = F ( x ) a = F ( b ) - F ( a ) where F ( x ) = ò f ( x ) dx
b
If f ( x ) ³ g ( x ) on a £ x £ b then Common Integrals Polynomials
ò dx = x + c
ó 1 dx = ln x + c ô õx
ò k dx = k x + c òx
-1
ò x dx = n + 1 x
n
1
n +1
+ c, n ¹ -1
Common Derivatives and Integrals
Inverse Trig Functions 1 ó æuö du = sin -1 ç ÷ + c ô èaø õ a2 - u2 1 ó 1 æuö du = tan -1 ç ÷ + c ô 2 2 a õ a +u èaø 1 1 ó æuö du = sec -1 ç ÷ + c ô 2 2 a èaø õ u u -a Hyperbolic Trig Functions ò sinh u du = cosh u + c
Miscellaneous ó 1 du = 1 ln u + a + c ô 2 õ a - u2 2a u - a
ò cosh u du = sinh u + c ò sech ò csch coth u du = - csch u + c ò csch ò sech u du = tan sinh u + c
-1
2 2
u du = tanh u + c u du = - coth u + c
ó 1 du = 1 ln u - a + c ô 2 õ u - a2 2a u + a
ò ò ò ò
u 2 a2 2 a + u du = a + u + ln u + a 2 + u 2 + c 2 2 u 2 a2 u 2 - a 2 du = u - a 2 - ln u + u 2 - a 2 + c 2 2 u 2 a2 æuö a 2 - u 2 du = a - u 2 + sin -1 ç ÷ + c 2 2 èaø
Given
b
Integration by Parts The standard formulas for integration by parts are,
ò udv = uv - ò vdu
òa
b
udv = uv a - ò vdu
a
b
b
Choose u and dv and then compute du by differentiating u and compute v by using the fact that v = ò dv .
ò a f ( g ( x ) ) g ¢ ( x ) dx then the substitution u = g ( x ) will convert this into the b g (b) integral, ò f ( g ( x ) ) g ¢ ( x ) dx = ò f ( u ) du . a g (a)
+c
ò sin u du = - cos u + c ò sec u du = tan u + c ò sec u tan u du = sec u + c ò csc u cot udu = - csc u + c ò csc u du = - cot u + c ò tan u du = ln sec u + c ò cot u du = ln sin u + c 1 ò sec u du = ln sec u + tan u + c ò sec u du = 2 ( sec u tan u + ln sec u + tan u ) + c
2 2
2au - u 2 du =
u-a a2 æ a -u ö 2au - u 2 + cos -1 ç ÷+c 2 2 è a ø
Standard Integration Techniques Note that all but the first one of these tend to be taught in a Calculus II class. u Substitution
© 2005 Paul Dawkins
Visit for a complete set of Calculus I & II notes.
Common Derivatives and Integrals
Trig Substitutions If the integral contains the following root use the given substitution and formula. a a2 - b2 x2 x = sin q Þ and cos 2 q = 1 - sin 2 q b a b2 x2 - a2 Þ x = sec q and tan 2 q = sec2 q - 1 b a a2 + b2 x2 Þ x = tan q and sec 2 q = 1 + tan 2 q b Partial Fractions ó P ( x) dx where the degree (largest exponent) of P ( x ) is smaller than the If integrating ô õ Q ( x)
