博弈论试题1
博弈论综合测试1-8

综合测试(一)一、填空题1.思维的逻辑形式是由( )和( )组成的。
在“如果p,那么q”中,“p”和“q”是( )项,“如果,那么”是( )。
2.由属概念过渡到种概念的逻辑方法是( );由种概念过渡到属概念的逻辑方法是( )。
3.一个命题的主项和谓项都周延,则这个命题是( )命题,一个命题的主项和谓项都不周延,则这个命题是( )命题。
4.当SAP假时,S与P的外延关系可能是( )关系、( )关系或( )关系。
5.我跑遍了杭州所有的书店都没有买到这本书,最后在“高教”书店才买到。
这句话违反了( )律的要求。
6.根据对当关系,SEP真,能推出“并非SAP”( ),SIP( )。
7.若有效三段论的前提中有O命题,则该三段论要么是第( )格,要么是第( )格。
8.若以“p←(┐q∨┐r)”和“┐(q∧r)”为前提进行假言推理,能必然推出结论。
二、单项选择题1.学校可以划分为大学、中学、小学和体育学校、业余学校。
这句话犯的划分错误是()。
A.多出子项B.子项相容C.概念含混D.划分不全2.若SOP为假,S与P的外延关系是()。
A.全同关系B.全异关系C.属种关系D.交叉关系3.“北京在上海的北面”和“北京是大城市”这两个命题()。
A.两个都是关系命题B.两个都是性质命题C.前者是关系命题,后者是性质命题D.前者是性质命题,后者是关系命题4.根据矛盾律的要求,若说“他既懂物理学又懂化学”,则不能说()。
A.“他或者懂物理学,或者懂化学”B.“并非他既懂物理学又懂化学”C.“他或者不懂物理学,或者懂化学”D.“他或者懂物理学,或者不懂化学”5.由PIS可以推出()。
A.SEP B.PES C.并非SEP D.PAS6.下列推理形式正确的是()。
A.p→q B.p→┐q ┐p所以q ┐q所以pC.┐p∨q D.p∨┐q∨r p所以q p∨r所以┐q7.由“如果p则q,如果r则s,p或r”,可推出()。
A.q∨s B.┐q∨┐s C.┐q∨s D.q∨┐s8.从“凡是正确的推理都是形式有效的推理”可以得出()。
博弈论考试题及答案

博弈论考试题及答案一、单项选择题(每题2分,共10题,共20分)1. 博弈论中,参与者在决策时不知道其他参与者的选择,这种博弈类型被称为:A. 完全信息博弈B. 不完全信息博弈C. 零和博弈D. 非零和博弈答案:B2. 在博弈论中,以下哪个概念描述的是参与者在博弈中所追求的目标?A. 纳什均衡B. 帕累托最优C. 占优策略D. 博弈收益答案:D3. 囚徒困境中,两个参与者如果都选择合作,他们将获得的收益是:A. 最大的B. 最小的C. 中等的D. 不确定的答案:A4. 以下哪个选项不是博弈论中的均衡概念?A. 纳什均衡B. 子博弈完美均衡C. 贝叶斯均衡答案:D5. 在博弈论中,如果一个策略在任何情况下都是最优的,那么这个策略被称为:A. 占优策略B. 纳什均衡C. 帕累托最优D. 混合策略答案:A6. 博弈论中的“混合策略”是指:A. 参与者随机选择纯策略B. 参与者总是选择相同的策略C. 参与者只选择一种策略D. 参与者不进行策略选择答案:A7. 在博弈论中,如果一个参与者的策略选择不依赖于其他参与者的策略选择,这种策略被称为:A. 占优策略B. 独立策略C. 混合策略D. 纳什均衡答案:A8. 博弈论中,以下哪个概念描述的是所有参与者都不可能通过单方面改变策略来提高自己的收益?A. 帕累托最优B. 纳什均衡C. 占优策略答案:B9. 在博弈论中,如果一个参与者的策略在其他所有参与者的策略给定时是最优的,这种策略被称为:A. 占优策略B. 纳什均衡C. 最优反应D. 混合策略答案:C10. 博弈论中的“动态博弈”是指:A. 参与者同时做出决策的博弈B. 参与者按顺序做出决策的博弈C. 参与者只进行一次决策的博弈D. 参与者不进行决策的博弈答案:B二、多项选择题(每题3分,共5题,共15分)1. 以下哪些是博弈论中的基本类型?A. 合作博弈B. 非合作博弈C. 完全信息博弈D. 不完全信息博弈答案:A, B, C, D2. 在博弈论中,以下哪些是描述均衡的概念?A. 纳什均衡B. 帕累托最优C. 占优策略均衡D. 混合策略均衡答案:A, C, D3. 以下哪些是博弈论中可能的结果?A. 帕累托最优B. 纳什均衡C. 占优策略均衡D. 混合策略均衡答案:A, B, C, D4. 在博弈论中,以下哪些是描述策略的概念?A. 纯策略B. 混合策略C. 占优策略D. 最优反应答案:A, B, C, D5. 以下哪些是博弈论中可能的决策顺序?A. 同时决策B. 顺序决策C. 重复决策D. 单次决策答案:A, B, C, D三、简答题(每题5分,共2题,共10分)1. 请简述博弈论中的“纳什均衡”是什么?答案:纳什均衡是指在一个博弈中,每个参与者都选择了自己的最优策略,并且这些策略在其他参与者的策略给定时是最优的,没有任何一个参与者可以通过单方面改变策略来提高自己的收益。
博弈论期末考试试题及答案

博弈论期末考试试题及答案# 博弈论期末考试试题及答案一、选择题(每题2分,共20分)1. 博弈论中,参与者在没有沟通的情况下进行决策,这种博弈被称为:A. 完全信息博弈B. 不完全信息博弈C. 零和博弈D. 非零和博弈答案:B2. 纳什均衡是博弈论中的一个概念,它描述了一种什么样的状态?A. 所有参与者都获得最大收益的状态B. 至少有一个参与者能获得更大收益的状态C. 没有参与者能通过单方面改变策略来获得更大收益的状态D. 所有参与者都获得相同收益的状态答案:C3. 以下哪个不是博弈论中的策略类型?A. 纯策略B. 混合策略C. 随机策略D. 确定性策略答案:D4. 博弈论中的囚徒困境指的是:A. 参与者合作可以获得最优结果B. 参与者背叛可以获得最优结果C. 参与者合作可以获得次优结果,但背叛可以获得最优结果D. 参与者背叛可以获得次优结果,但合作可以获得最优结果答案:C5. 以下哪个不是博弈论中的基本概念?A. 参与者B. 策略C. 收益D. 概率答案:D...二、简答题(每题10分,共30分)1. 解释什么是博弈论,并给出一个实际生活中的例子。
答案:博弈论是研究具有冲突和合作特征的决策者之间互动的数学理论。
在实际生活中,博弈论的一个例子是拍卖。
在拍卖中,买家(参与者)需要决定出价(策略)以赢得商品(收益),同时考虑其他买家的出价策略。
2. 描述纳什均衡的概念,并解释为什么它在博弈论中如此重要。
答案:纳什均衡是指在非合作博弈中,每个参与者选择自己的最优策略,并且考虑到其他参与者的策略选择时,没有参与者能通过单方面改变策略来获得更大的收益。
纳什均衡在博弈论中非常重要,因为它提供了一种预测参与者行为的方法,即在均衡状态下,参与者没有动机去改变他们的策略。
3. 什么是完全信息博弈和不完全信息博弈?它们之间有什么区别?答案:完全信息博弈是指所有参与者都完全知道博弈的结构和其他参与者的收益函数。
而不完全信息博弈是指至少有一个参与者对博弈的结构或其它参与者的收益函数不完全了解。
博弈论复习题(1)

1.设一四阶段两博弈方之间的动态博弈如图所示。
试找出全部子博弈,讨论该博弈中的可信性问题,求子博弈完美纳什均衡策略组合和博弈的结果。
2.假设一个工会是一个寡头垄断市场中所有企业唯一的劳动力供给者,就像汽车工人联合会对于通用、福特、克莱斯勒等大的汽车厂家。
令博弈各方行动的时间顺序如下:(1)工会确定单一的工资要求w ,适用于所有的企业(2)每家企业i 了解到w ,然后同时分别选择各自的雇佣水平L i ;(3)工会的收益为(w-w α)L ,其中w α为工会成员到另外的行业谋职可取得的收入,L=L 1+…L n 为工会在本行业企业的总就业水平;企业i 的利润为π(w ,L i ),其中决定企业i 利润水ABB A h g (2,4)(8,5)(3,6)(4,3)b (5,3)a c d f e平的要素如下。
所有企业都有同样的生产函数:产出等于劳动力q i=L i。
市场总产出为Q=q1+…+q n时的市场出清价格为p(Q)=a-Q。
为使问题简化,假设企业除了工资支出外没有另外的资本。
求出此博弈的子博弈精炼解。
在子博弈精炼解中,企业的数量是如何影响工会的效应的?为什么?(吉本斯2.2节 2.7答案)3.下图所示的同时行动博弈重复进行两次,并且第二阶段开始前双方可观测到第一阶段的结果,不考虑贴现因素。
变量x大于4,因而(4,4)在一次性博弈中并不是一个均衡收益。
对什么样的x,(双方参与者同时采取)下述战略是一个子博弈完美纳什均衡?第一阶段选择Q i,如果第一阶段的结果为(Q1,Q2),在第二阶段选择P i;如果第一阶段的结果为(y,Q2),其中y≠Q1,第二阶段选择R i;如果第一阶段的结果为(Q1,z),其中z≠Q1,第二阶段选择S i;如果第一阶段结果为(y,z),其中y≠Q1,且z≠Q2,则在第二阶段选P iP2 Q2 R2 S2P1Q1R1S1(2.10吉本斯)思路:逐个分析上述的四种情形:第一种情形,第一阶段选择Qi,第二阶段选择Pi,即双方均采取合作的策略,得益均为6;第二种情形和第三种情形下,实际上有一方是采取了不合作,其得益为x,另一方即利益受损方得益为2;第四种情形实际上是双方都不采取合作的策略,而根据题目要求,对于x,下述战略是一个子博弈精炼纳什均衡,所以x必须小于双方均合作时的收益6,否则第一种情形不会出现,因为既然x>6了,双方均会选择不合作而使情形一不会出现。
博弈论习题[1]
![博弈论习题[1]](https://img.taocdn.com/s3/m/20aa7d03de80d4d8d15a4ff9.png)
习题 2
解: 令(L,M,R)是参与人 A 的 战 略 ;( U,M,D)是参与人 B 的战略。从表 1A.2 中可以看出,对 于参与人 B 而言,M 战略是严格劣于 R 战略,按照重复提出的占有均衡剔除 M 战略,则得出 如表(a)博弈。在表(a)博弈,参与人 A 的 M 和 D 战略严格劣于 U 战略,重复剔除 M 和 D 战略,得出表(b)博弈。该博弈为单人决策,则重复剔除的占优均衡为(U,L)。
r3 ( A, A, A) = r3 ( A, A, B) = r3 (A, A, C) = {A, B, C} r3 ( A, B, A) = r3 ( A, B, B) = r3 (A, B, C) = {A, C} r3 ( A, C, A) = r3 ( A, C, B) = r3 ( A, C, C) = {C} r3 (B, A, A) = r3 (B, A, B) = r3 (B, A, C) = {A, C} r3 (B, B, A) = r3 (B, B, B) = r3 (B, B, C) = {B, C} r3 (B, C, A) = r3 (B, C, B) = r3 (B, C, C) = {C} r3 (C, A, A) = r3 (C, A, B) = r3 (C, A, C) = {C} r3 (C, B, A) = r3 (C, B, B) = r3 (C, B, C) = {C} r3 (C, C, A) = r3 (C, C, B) = r3 (C, C, C) = {A, B, C}
(0,0)律师得到 100 (0,0)律师得到 100)
通过上面的支付矩阵,我们可以得出答案,只有 A 和 B 都做出选择得到 50 的时候,他
们才能得到钱,不多一分也不少分,如果有一方想多得,二者将一分也得不到,钱全部归律
“博弈论”习题及参考答案

《博弈论》习题一、单项选择题1.博弈论中,局中人从一个博弈中得到的结果常被称为()。
A. 效用B. 支付C. 决策D. 利润2.博弈中通常包括下面的内容,除了()。
A.局中人B.占优战略均衡C.策略D.支付3.在具有占优战略均衡的囚徒困境博弈中()。
A.只有一个囚徒会坦白B.两个囚徒都没有坦白C.两个囚徒都会坦白D.任何坦白都被法庭否决了4.在多次重复的双头博弈中,每一个博弈者努力()。
A.使行业的总利润达到最大B.使另一个博弈者的利润最小C.使其市场份额最大D.使其利润最大5.一个博弈中,直接决定局中人支付的因素是()。
A. 策略组合B. 策略C. 信息D. 行动6.对博弈中的每一个博弈者而言,无论对手作何选择,其总是拥有惟一最佳行为,此时的博弈具有()。
A.囚徒困境式的均衡B.一报还一报的均衡C.占优策略均衡D.激发战略均衡7.如果另一个博弈者在前一期合作,博弈者就在现期合作;但如果另一个博弈者在前一期违约,博弈者在现期也违约的策略称为()。
A.一报还一报的策略B.激发策略C.双头策略D.主导企业策略8.在囚徒困境的博弈中,合作策略会导致()。
A.博弈双方都获胜B.博弈双方都失败C.使得先采取行动者获胜D.使得后采取行动者获胜9.在什么时候,囚徒困境式博弈均衡最可能实现()。
A. 当一个垄断竞争行业是由一个主导企业控制时B.当一个寡头行业面对的是重复博弈时C.当一个垄断行业被迫重复地与一个寡头行业博弈时D. 当一个寡头行业进行一次博弈时10.一个企业采取的行为与另一个企业在前一阶段采取的行为一致,这种策略是一种()。
A.主导策略B.激发策略C.一报还一报策略D.主导策略11.关于策略式博弈,正确的说法是()。
A. 策略式博弈无法刻划动态博弈B. 策略式博弈无法表明行动顺序C. 策略式博弈更容易求解D. 策略式博弈就是一个支付矩阵12.下列关于策略的叙述哪个是错误的():A. 策略是局中人选择的一套行动计划;B. 参与博弈的每一个局中人都有若干个策略;C. 一个局中人在原博弈中的策略和在子博弈中的策略是相同的;D. 策略与行动是两个不同的概念,策略是行动的规则,而不是行动本身。
博弈数学测试题及答案

博弈数学测试题及答案一、选择题(每题2分,共20分)1. 以下哪个不是博弈论中的基本概念?A. 纳什均衡B. 混合策略C. 马尔可夫链D. 纯策略答案:C2. 在零和博弈中,以下哪个说法是正确的?A. 参与者的收益总和为零B. 参与者的损失总和为零C. 参与者的总收益为零D. 参与者的总损失为零答案:A3. 以下哪个不是博弈论中的策略类型?A. 纯策略B. 混合策略C. 静态策略D. 动态策略答案:D4. 博弈论中的“囚徒困境”主要说明了什么?A. 合作总是最优选择B. 个体理性可能导致集体非理性C. 集体理性总是最优选择D. 个体非理性可能导致集体理性答案:B5. 在博弈论中,以下哪个不是纳什均衡的特点?A. 每个参与者都选择了自己的最优策略B. 每个参与者的策略都是对其他参与者策略的最佳响应C. 参与者可以通过改变策略来获得更好的结果D. 所有参与者都达到了自己可能的最大收益答案:C6. 以下哪个是博弈论中的合作博弈?A. 囚徒困境B. 石头剪刀布C. 公共品博弈D. 零和博弈答案:C7. 在博弈论中,哪个术语描述了参与者在没有沟通的情况下做出决策?A. 沟通博弈B. 非合作博弈C. 同时博弈D. 顺序博弈答案:C8. 以下哪个不是博弈论中的结果类型?A. 帕累托效率B. 纳什均衡C. 社会福利最大化D. 个人最优答案:D9. 在博弈论中,以下哪个不是博弈的分类?A. 完全信息博弈B. 不完全信息博弈C. 静态博弈D. 动态博弈答案:C10. 以下哪个是博弈论中的“重复博弈”?A. 参与者只进行一次决策B. 参与者进行多次决策C. 参与者在博弈中没有记忆D. 参与者在博弈中不能交流答案:B二、填空题(每空1分,共10分)1. 博弈论是由数学家______提出的。
答案:约翰·冯·诺伊曼2. 博弈论中的“纳什均衡”是由______命名的。
答案:约翰·纳什3. 在博弈论中,如果参与者的策略选择是相互独立的,这种博弈被称为______博弈。
博弈论十五道题以及答案

博弈论十五道题以及答案1.博弈理论在哪些方面扩展了传统的新古典经济学?2.法律和信誉是维持市场有序运行的两个基本机制。
请结合重复博弈理论谈谈信誉机制发生作用的几个条件。
3.经济发展史表明,在本来不认识的人之间建立相互之间的信任关系是经济发展的关键。
为什么?4.在传统社会中,即使没有法律,村民之间也可以建立起高度的信任。
请结合博弈理论解释其原因。
5.在旅游地很容易出现假货,而在居民小区的便利店则很少出现假货,请结合博弈论的相关理论进行解释。
6.你如何理解“Credible threats or promises about future behavior can influence current behavior”这句话的?7.有效的法律制度对经济发展具有什么作用?请结合博弈理论谈谈你的理解。
8.试用博弈理论解释家族企业为什么难以实行制度化管理?9.固定资产投资为什么可以作为一种可置信的承诺?10.以汽车保险为例谈谈因为信息不对称所可能产生的道德风险问题,并提出一种解决道德风险的方案。
11.以公司为例,谈谈所有者与经营者的分离可能产生的道德风险问题。
12.在波纳佩岛上,谁能种出特别大的山药,谁的社会地位就高,谁就能赢得人们的尊敬并可担任公共职务。
请结合信号传递模型谈谈波纳佩岛上的这种奇异风俗。
13.一位男生在女朋友过生日时送给女朋友三百元人民币,他的女朋友往往感觉受到了侮辱。
而他女朋友可能会欣然接受父母亲的现金礼物。
请解释其中可能的原因。
14.<圣经>(旧约)中记载了两个母亲争夺一个孩子的故事。
一次,两个女人为争夺一个婴儿争扯到所罗门王殿前,她们都说婴儿是自己的,请所罗门王作主。
所罗门王稍加思考后作出决定:将婴儿一刀劈为两段,两位妇人各得一半。
这时,其中一位妇人立即要求所罗门王将婴儿判给对方,并说婴儿不是自己的,应完整归还给另一位妇人,千万别将婴儿劈成两半。
听罢这位妇人的求诉,所罗门王立即作出最终裁决——婴儿是这位请求不杀婴儿的妇人的,应归于她。
博弈论练习题 第一组 参考答案
4
6.一个支付组合是帕累托有效率的,当且仅当没有任何其他的支付组合可以同时 改善所有人的处境。假定A和B两人组成一个社会,可能的支付组合如下:
组合1(200,200),组合2(0,300),组合3(300,0),组合4(100,100), (这里(200,200)表示A的支付为200,B的支付为200)。 (1)假定只有如上可能的四个支付组合。找出下列支付组合中帕累托有效率的
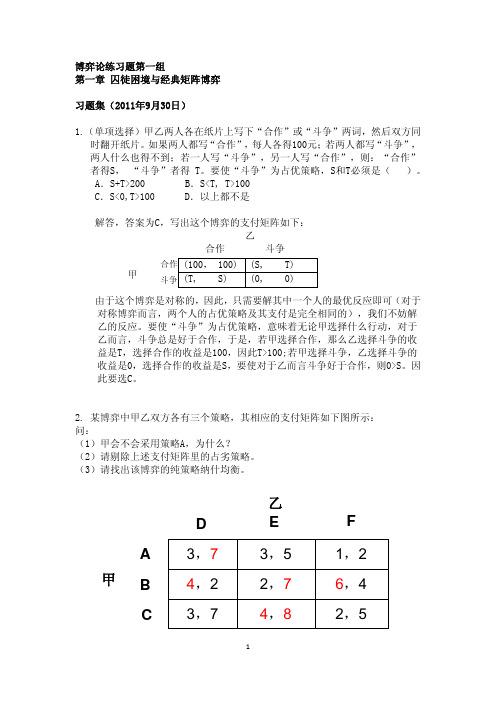
2. 某博弈中甲乙双方各有三个策略,其相应的支付矩阵如下图所示: 问: (1)甲会不会采用策略A,为什么? (2)请剔除上述支付矩阵里的占劣策略。 (3)请找出该博弈的纯策略纳什均衡。
A 甲B
C
D 3,7 4,2 3,7
乙 E
3,5 2,7 4,8
F 1,2 6,4 2,5
1
答案:1)甲不会采用策略A,策略A是甲的劣策略,它是劣于C的。 2)对于甲而言,A是一个劣策略。对于乙而言,F是一个劣策略(做到这一步即
A.S+T>200
B.S<T, T>100
C.S<0,T>100
D.以上都不是
解答,答案为C,写出这个博弈的支付矩阵如下:
乙
合作
斗争
合作 (100, 100) (S, T) 甲 斗争 (T, S) (0, 0)
由于这个博弈是对称的,因此,只需要解其中一个人的最优反应即可(对于 对称博弈而言,两个人的占优策略及其支付是完全相同的),我们不妨解 乙的反应。要使“斗争”为占优策略,意味着无论甲选择什么行动,对于 乙而言,斗争总是好于合作,于是,若甲选择合作,那么乙选择斗争的收 益是T,选择合作的收益是100,因此T>100;若甲选择斗争,乙选择斗争的 收益是0,选择合作的收益是S,要使对于乙而言斗争好于合作,则0>S。因 此要选C。
博弈论习题1

1、一逃犯从关押他的监狱中逃走,一看守奉命追捕。
如果逃
犯逃跑有两条可选择的路线,看守只要追捕方向正确就一定能抓住逃犯。
逃犯逃脱可少坐10年牢,但一旦被抓住则要
加刑10年;看守抓住逃犯能得1000元奖金。
请用得益矩阵表示该博弈。
2、你正在考虑是否投资100万元开设一家饭店。
假设情况是
这样的:你决定开,则0.35的概率你将收益300万元(包
括投资),而0.65的概率你将全部亏损掉;如果你不开,
则你能保住本钱但也不会有利润。
请你:
(1)用得益矩阵表示该博弈。
(2)如果你是风险中性者,你会怎样选择?
(3)如果你是风险规避者,且期望得益的折扣系数为0.9,你的策略选择是什么?
3、假设长虹和创维这两家彩电生产商都可以选择集中力量研
发生产低档产品或高档产品,但他们在选择时都不知道对方的选择。
假设他们在不同选择下的收益矩阵如下图所示。
请问:该博弈的均衡是什么?。
博弈论试题及答案

博弈论试题及答案【正文】博弈论试题及答案一、选择题1.博弈论是研究:A. 地理分布B. 人类视力C. 决策制定D. 古典文学答案:C2.下列哪个不是博弈论中常见的概念?A. 纳什均衡B. 优势策略C. 输家效应D. 零和博弈答案:C3.描述纳什均衡的最佳方式是:A. 所有参与者都达到最佳策略B. 至少有一个参与者达到最佳策略C. 所有参与者都达到次优策略D. 至少有一个参与者达到次优策略答案:A4.下列哪个案例体现了零和博弈的情况?A. 两国签订贸易协定B. 赌徒在赌博中争夺赌注C. 两家公司合作推出新产品D. 好友一起玩棋盘游戏答案:B5.下列哪个不是博弈论的应用之一?A. 经济决策B. 政治博弈C. 生物进化D. 音乐创作答案:D二、填空题1.博弈论最早由_____________等人于20世纪40年代提出。
答案:冯·诺依曼(John von Neumann)2.博弈论是研究参与者间的_____________和_____________的学科。
答案:互动行为;决策制定3.零和博弈是指参与者的利益总和恒为_____________。
答案:零4.博弈论中的最佳策略指的是在其他参与者采取某个策略时,使某一参与者的_____________最大化的策略。
答案:利益5.斯坦福大学的_____________教授以其对博弈论的突出贡献而获得2005年诺贝尔经济学奖。
答案:约翰·纳什(John Nash)三、简答题1.简要解释博弈论中的纳什均衡。
答:纳什均衡是博弈论中的一个重要概念,指的是在参与者选择自己最佳策略的情况下,不存在任何一个参与者可以通过单独改变自己的策略来获得更好收益的状态。
简言之,纳什均衡是一种理性选择下的稳定状态。
2.举例说明博弈论在实际生活中的应用。
答:博弈论在经济学、政治学、生物学等领域中都有广泛应用。
例如,在贸易谈判中,两个国家之间的博弈就是典型的博弈论应用。
博弈论考试题及答案

博弈论考试题及答案一、选择题(每题2分,共20分)1. 博弈论中的“囚徒困境”是指什么?A. 两个囚犯相互合作B. 两个囚犯相互背叛C. 两个囚犯中一个合作一个背叛D. 两个囚犯相互猜疑答案:B2. 以下哪个不是博弈论中的基本概念?A. 策略B. 收益C. 公平D. 纳什均衡答案:C3. 在零和博弈中,一个玩家的损失等于另一个玩家的收益,这意味着:A. 总收益为零B. 总收益为正C. 总收益为负D. 总收益不确定答案:A4. 博弈论中的“混合策略”是指:A. 玩家随机选择策略B. 玩家固定选择一种策略C. 玩家根据对手的策略选择策略D. 玩家不使用策略答案:A5. 以下哪个是博弈论中的“完全信息”博弈?A. 拍卖博弈B. 石头剪刀布C. 桥牌D. 信息不对称博弈答案:C6. 博弈论中的“重复博弈”指的是:A. 博弈只进行一次B. 博弈进行多次C. 博弈进行无限次D. 博弈进行有限次但次数未知答案:B7. 以下哪个是博弈论中的“动态博弈”?A. 零和博弈B. 非零和博弈C. 同时博弈D. 顺序博弈答案:D8. 在博弈论中,如果一个策略组合是纳什均衡,那么:A. 每个玩家都有动机单方面改变策略B. 每个玩家都满足于当前策略C. 至少有一个玩家不满意当前策略D. 所有玩家都不满意当前策略答案:B9. 博弈论中的“合作博弈”是指:A. 玩家之间可以形成联盟B. 玩家之间不能形成联盟C. 玩家之间只能通过竞争来获得收益D. 玩家之间只能通过合作来获得收益答案:A10. 以下哪个是博弈论中的“公共知识”?A. 每个玩家的收益函数B. 每个玩家的策略选择C. 每个玩家的偏好D. 每个玩家的个人信息答案:A二、简答题(每题10分,共30分)1. 简述博弈论中的“纳什均衡”概念。
答案:纳什均衡是指在一个博弈中,每个玩家都选择了自己的最优策略,并且没有玩家能够通过单方面改变策略来提高自己的收益。
在纳什均衡状态下,每个玩家的策略是对其他玩家策略的最优反应。
经济博弈论试题及答案

经济博弈论试题及答案一、选择题(每题2分,共20分)1. 博弈论中的“纳什均衡”是由哪位经济学家提出的?A. 亚当·斯密B. 约翰·纳什C. 保罗·萨缪尔森D. 米尔顿·弗里德曼答案:B2. 在完全信息博弈中,参与者对其他参与者的策略和收益函数了解多少?A. 完全不了解B. 部分了解C. 完全了解D. 只了解自己的收益函数答案:C3. 以下哪个是博弈论中的基本概念?A. 边际成本B. 边际效用C. 策略组合D. 机会成本答案:C4. 囚徒困境中,如果两个囚徒都选择不合作,他们将面临什么结果?A. 获得最大利益B. 获得最小利益C. 获得中等利益D. 无法预测答案:B5. 以下哪个不是博弈论中的策略类型?A. 纯策略B. 混合策略C. 随机策略D. 固定策略答案:D二、简答题(每题10分,共30分)6. 简述博弈论在经济学中的应用。
答案:博弈论在经济学中的应用广泛,包括但不限于市场结构分析、拍卖理论、竞争策略、合作与联盟形成、公共品提供、以及政策制定等。
博弈论帮助经济学家理解个体在不同情境下的决策行为,预测市场动态,为政策制定提供理论依据。
7. 解释什么是“零和博弈”。
答案:零和博弈是一种特殊类型的博弈,其中参与者的总收益为零。
这意味着一个参与者的收益增加必然伴随着另一个参与者的损失,整个博弈的总收益是不变的。
这种博弈通常涉及到竞争关系,而非合作。
8. 描述“重复博弈”的特点。
答案:重复博弈是指同样的博弈结构在一定时间间隔内重复进行多次。
其特点包括参与者可以学习对手的行为模式,策略可能随时间调整,以及长期合作的可能性增加。
重复博弈中的策略选择往往更加复杂,因为参与者需要考虑未来博弈的影响。
三、计算题(每题25分,共50分)9. 假设有两个公司A和B,它们可以选择高成本生产(H)或低成本生产(L)。
如果两个公司都选择H,它们各自获得利润为100万元;如果都选择L,各自获得利润为50万元;如果一个选择H而另一个选择L,选择L的公司获得150万元利润,而选择H的公司则亏损50万元。
博弈论初步(1)

【例题1.1.1】出硬币博弈———硬币配对游戏
解甲、乙两人各拿一枚1元硬币,背靠背各自将硬币正面(1元币值面)或反向(菊花图案面)朝上置于手掌心中握紧。
然后,转身面对面,同时展开手掌。
游戏规则是,如果两人掌上硬币都是正面朝上或都是反面朝上,那么甲就赢了乙1元钱,或者说乙输给了甲1元钱;如果两人掌上硬币是一正一反,那么乙就赢了甲1元钱,或者说甲输给了乙1元钱。
【例题1.1.2】价格竞争博弈
解企业A与企业B生产同一种商品在市场上形成双寡头垄断市场,它们对商品都可以选择三种价格(高价、中价或低价)投放市场。
市场规则:不管哪个企业开出较低价格,它就可以得整个市场;如果两个企业开价相同,它们将平分市场。
这里进一步给出企业A,B选择不同价格获得的收益情况:企业A选择“中价”,企业B选择“高价”,这时企业A独占市场收益10万元;企业A选择“低价”,而企业B选择“高价”或“中价”,这时企业A仍独占市场,但因定出“低价”,因此收益为8万元。
当企业B选择较低价格,情况类同。
当企业A,B同时都选择“高价”,这时它们平分市场,各自收益为6万元;同时都选择“中价”,各自收益为5万元;同时都选择“低价”,各自收益为4万元。
博弈论复习题及答案

博弈论复习题及答案1. 博弈论中,非合作博弈与合作博弈的主要区别是什么?答案:非合作博弈是指参与者之间没有约束性协议的博弈,每个参与者都独立地选择自己的策略以最大化自己的利益。
而合作博弈则允许参与者之间形成具有约束力的协议,共同合作以达到共同的目标。
2. 什么是纳什均衡?答案:纳什均衡是指在一个博弈中,每个参与者都选择了最优策略,并且考虑到其他参与者的策略后,没有参与者有动机单方面改变自己的策略。
3. 零和博弈与非零和博弈有何不同?答案:零和博弈是指博弈中所有参与者的收益总和为零,即一个参与者的收益必然导致另一个参与者的损失。
非零和博弈则是指参与者的收益总和不为零,参与者之间可能存在合作共赢的情况。
4. 如何判断一个博弈是否存在纯策略纳什均衡?答案:可以通过构建博弈的收益矩阵,然后寻找每个参与者在其他参与者策略给定的情况下的最佳响应策略。
如果存在一组策略,使得每个参与者在其他参与者策略不变的情况下,都没有动机改变自己的策略,那么这个策略组合就是一个纯策略纳什均衡。
5. 混合策略纳什均衡与纯策略纳什均衡有何不同?答案:纯策略纳什均衡是指参与者在均衡状态下选择的策略是确定的,而混合策略纳什均衡则是指参与者在均衡状态下选择的策略是随机的,每个策略都有一定的概率被选择。
6. 什么是支配策略?答案:支配策略是指在博弈中,无论其他参与者选择什么策略,某个参与者选择该策略都能获得比其他策略更好的结果。
7. 博弈论中的“囚徒困境”说明了什么?答案:“囚徒困境”说明了即使合作对所有参与者都有利,但由于缺乏信任和沟通,参与者可能会选择对自身最有利的策略,导致集体结果不是最优的。
8. 什么是博弈论中的“倒后归纳法”?答案:“倒后归纳法”是一种解决动态博弈的方法,通过从博弈的最后阶段开始,逆向分析每个阶段的最优策略,直到博弈的初始阶段。
9. 博弈论在经济学中的应用有哪些?答案:博弈论在经济学中的应用非常广泛,包括但不限于市场结构分析、拍卖理论、合同理论、产业组织、宏观经济政策分析等。
博弈论复习题及答案

博弈论复习题及答案博弈论是研究决策者在相互影响的情况下如何做出决策的理论。
以下是一些博弈论的复习题及答案,供学习者参考:一、选择题1. 博弈论中最基本的博弈类型是什么?A. 零和博弈B. 非零和博弈C. 完全信息博弈D. 不完全信息博弈答案: A. 零和博弈2. 在博弈论中,纳什均衡指的是什么?A. 一种博弈的最终结果B. 一种博弈的初始状态C. 一种策略组合,其中没有任何一个参与者能够通过单方面改变策略而获得更好的结果D. 一种策略组合,其中所有参与者都希望改变策略以获得更好的结果答案: C. 一种策略组合,其中没有任何一个参与者能够通过单方面改变策略而获得更好的结果3. 囚徒困境中,两个囚犯的最优策略是什么?A. 相互合作B. 相互背叛C. 一个合作,一个背叛D. 一个背叛,一个合作答案: B. 相互背叛二、简答题1. 解释什么是“混合策略”并给出一个例子。
答案:混合策略是指参与者在博弈中选择不同策略的概率分布。
例如,在石头、剪刀、布的游戏中,一个玩家可能会以1/3的概率选择石头,1/3的概率选择剪刀,和1/3的概率选择布,这就是一个混合策略的例子。
2. 描述什么是“重复博弈”以及它与单次博弈的区别。
答案:重复博弈是指同样的博弈结构在一定时间内多次进行。
与单次博弈相比,重复博弈允许参与者根据对手过去的行动来调整自己的策略,这可能会导致合作行为的出现,因为参与者会考虑到未来博弈的潜在收益。
三、论述题1. 论述博弈论在经济学中的应用,并给出至少两个具体的例子。
答案:博弈论在经济学中有广泛的应用。
例如:- 拍卖理论:博弈论可以用来分析拍卖中的竞价行为,确定最优的拍卖机制。
- 竞争策略:企业在制定市场进入、定价和广告策略时,会使用博弈论来预测竞争对手的行为并做出相应的决策。
2. 讨论囚徒困境在现实世界中可能的应用场景,并解释为什么合作有时是困难的。
答案:囚徒困境在现实世界中的应用场景包括但不限于:- 国际关系:国家之间的军备竞赛可以看作是囚徒困境的一种形式,合作减少军备可以带来共同的利益,但由于缺乏信任,每个国家都倾向于增加自己的军备。
经济博弈论试题及答案

经济博弈论试题及答案(正文部分)第一部分:试题1. 请简要解释什么是经济博弈论。
2. 请列举并解释博弈论中的一些重要概念,如纳什均衡、占优策略和囚徒困境等。
3. 在实际生活中,经济博弈论有哪些应用领域?请举例说明。
4. 什么是合作博弈?请阐述合作博弈的特点,并提供一个相关的实例。
5. 请简述零和博弈与非零和博弈的区别,并给出一个具体案例。
第二部分:答案1. 经济博弈论是一种集合数学、经济学和策略分析于一体的理论框架,用于研究决策者在相互关联的环境中做出决策时所面临的策略选择和结果影响。
2. (1) 纳什均衡:指在博弈中,所有参与者都选择最优策略时所构成的一组策略组合,使得没有一个参与者单方面改变策略可以使自己的收益提高。
(2) 占优策略:指在博弈中,一方参与者在某种策略下收益最大化,无论其他参与者采用何种策略。
(3) 囚徒困境:是博弈论中的一个经典案例,描述的是两个囚犯是否应该合作以最大化自己的收益。
在该案例中,即使合作能带来最优结果,囚犯之间因互相不信任而往往选择背叛。
3. 经济博弈论在实际生活中有广泛的应用。
例如:(1) 在企业竞争中,博弈论可以帮助企业决定定价策略和市场竞争策略,以及对手可能采取的行动。
(2) 在国际贸易谈判中,博弈论可以用于分析各个国家的利益诉求和谈判策略,以实现最优结果。
(3) 在环境保护领域,博弈论可以用于研究各个利益相关方之间的博弈行为,以促进合作与共识。
4. 合作博弈是指参与者在博弈中通过合作来实现收益最大化的行为。
合作博弈的特点包括:(1) 合作和沟通:参与者可以进行合作,共同制定策略,并通过沟通交流来实现最优结果。
(2) 利益共享:参与者之间共享合作所带来的利益,以实现总体收益的最大化。
(3) 长期合作:合作博弈通常需要参与者在长期内保持合作,以实现稳定的收益。
例子:两个企业在同一个市场上竞争,它们可以选择合作并共同制定定价策略,以实现最大化利润。
通过长期合作和有序竞争,两个企业可以避免价格战和利润损失。
《博弈论》期末考试试题

《博弈论》期末考试试题2003年12月,适用于:工商管理2000限选、全校2001任选1、鹰-鸽(Hawk-Dove)博弈(30分)两动物为某一食物而争斗。
每只动物都能象鸽或鹰那样行动。
对每只动物来说最坏的结果是两个都象鹰一样,此时的争斗使得双方都吃不到食物;如果两只动物合作起来象鸽一样行动,则每只动物都可吃到3个单位的食物;如果自己象鸽而对手象鹰,则自己只能吃到1个单位而对手可吃到4个单位。
假设两只动物进行的是一次性完全信息静态博弈,请回答如下问题:(1)请作出此博弈的支付矩阵,并明确描述出博弈的参与人和他的行动空间。
(2)请求解此博弈的全部纳什均衡(纯策略或混合策略纳什均衡)。
(3)请举一个现实生活中的例子并用鹰-鸽博弈进行解释。
2、狩猎博弈(20分)卢梭在他的《论人类不平等的起源和基础》中说到:如果一群猎人出发去猎一头鹿,他们完全意识到,为了成功,他们必须都要忠实地坚守自己的位置;然而如果一只野兔碰巧经过他们中的一个人附近,毫无疑问他会毫不迟疑地追逐它,一旦他获得了自己的猎物,他就不太关心他的同伴是否错失了他们的目标。
现在对上述描述简化。
假设只有两个猎人,他们必须同时决定是猎鹿还是野兔。
如果两个人均决定猎鹿,那么他们会获得一头鹿(价值1000元),并在他们之中进行平分;如果两个人均猎野兔,那么他们每个人可以获得一只野兔(价值100元);如果一个猎兔而另一个猎鹿,则前者获得一只野兔,后者将一无所获。
如果每个人都希望自己得到尽可能多的猎物,请作出支付矩阵并分析此博弈的纯策略纳什均衡。
3、设一四阶段两博弈方之间的动态博弈如下图所示,请回答下列问题。
(30分)(1)试找出全部子博弈;(2)讨论该博弈中的可信性问题;(3)求解子博弈精练纳什均衡和博弈的结果。
4、试运用所学知识解释下列现象(20分):根据经济学的基本原理,一般商品都是价格越低购买者越多,但为什么在现实生活中会出现低价不好销售、提高价格后反而更好销售的现象呢?你认为什么样的商品容易出现这种反常现象?。
博弈论经济学考试题及答案

博弈论经济学考试题及答案博弈论是经济学中的一个重要分支,它研究具有冲突和合作特征的决策者之间的互动。
以下是一套博弈论经济学的考试题及答案,供参考。
一、选择题(每题2分,共20分)1. 以下哪项不是博弈论的基本概念?A. 纳什均衡B. 完全信息C. 零和游戏D. 边际效用答案:D2. 囚徒困境中,如果两个囚犯都选择不合作,他们将各自获得什么结果?A. 最大利益B. 最小损失C. 纳什均衡D. 合作结果答案:C3. 以下哪个不是博弈论中的策略类型?A. 纯策略B. 混合策略C. 随机策略D. 确定性策略答案:D4. 博弈论中的“支配策略”指的是什么?A. 总是最优的策略B. 总是最差的策略C. 只在某些情况下最优的策略D. 只在对手采取特定策略时最优的策略答案:A5. 重复博弈中,以下哪个概念不是用于描述博弈的长期行为?A. 信誉B. 惩罚C. 一次性博弈D. 合作答案:C二、简答题(每题10分,共30分)1. 请简述什么是纳什均衡,并给出一个例子。
答案:纳什均衡是博弈论中的一个概念,指的是在一个非合作博弈中,每个参与者选择了自己的策略,并且没有一个人可以通过改变自己的策略而单方面获得更好的结果。
例如,在囚徒困境中,如果两个囚犯都选择沉默,那么他们各自获得的结果比互相揭发要好,即使他们知道如果都揭发对方,结果会更糟。
这种情况下,沉默就是他们的纳什均衡。
2. 解释“完全信息”和“不完全信息”博弈的区别。
答案:完全信息博弈是指所有参与者都完全知道博弈的结构和所有其他参与者的收益函数。
而不完全信息博弈是指至少有一个参与者对博弈的结构或至少一个其他参与者的收益函数不完全了解。
例如,在拍卖中,如果所有竞拍者都知道拍卖品的真实价值,这就是完全信息博弈;如果竞拍者对拍卖品的价值只有部分信息,这就是不完全信息博弈。
3. 什么是混合策略均衡?请举例说明。
答案:混合策略均衡是指在博弈中,参与者以一定的概率选择不同的策略,使得没有参与者可以通过改变自己的策略选择而获得更好的结果。
博弈论期末试题及答案

博弈论期末试题及答案一、选择题(每题2分,共40分)1. 博弈论的核心概念是:A. 均衡分析B. 策略分析C. 利润分析D. 收益分析2. Nash均衡是指:A. 所有玩家达到最优结果B. 没有玩家可以通过改变策略获得更好结果C. 所有玩家都选择相同的策略D. 所有玩家都选择不同的策略3. 在零和博弈中,一方的收益是另一方的:A. 收益的相反数B. 收益的平方C. 收益的负数D. 收益的倒数4. 最优响应策略是指:A. 在对手的策略给定时,玩家自己的最优策略B. 在对手的策略给定时,对手的最优策略C. 利用数学模型计算得到的最优策略D. 随机选择的策略5. 以下哪个是非合作博弈的扩展形式:A. 矩阵形式B. 博弈树形式C. 序列形式D. 重复博弈形式6. 当两位玩家在重复博弈中都选择合作策略时,他们的总收益是:A. 最大化的B. 最小化的C. 平均化的D. 不确定7. 最优子博弈在博弈树中的作用是:A. 寻找博弈的子集B. 确定博弈过程的时间C. 减少博弈的复杂性D. 避免剪枝8. 以下哪个是非合作博弈的解决概念:A. 纳什均衡B. 支配策略C. 策略剖析D. 相对策略9. 在纳什均衡中,每个玩家都是:A. 个体理性的B. 无知的C. 合作的D. 随机的10. 在博弈论中,支配策略指的是:A. 无论对手选择什么策略,都能带来最好结果的策略B. 无论自己选择什么策略,都能带来最好结果的策略C. 无论对手选择什么策略,都会带来最坏结果的策略D. 无论自己选择什么策略,都会带来最坏结果的策略二、简答题(每题10分,共20分)1. 请解释什么是零和博弈,并举例说明。
零和博弈是一种博弈模型,其中一个玩家的收益等于另一个玩家的损失,总收益为零,也就是说一方的利益必然导致另一方的损失。
举例来说,两个商家在一个市场上销售相同的商品,他们之间的竞争就可以看作是零和博弈。
一方的销售额的增加必然导致另一方的销售额减少。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Introduction to Game Theory Problem Set 1
Shanghai University of Finance and Economics
Professor Derek Tai-wei Liu
1. Player A offers player B a gamble. For a price, he would throw a dice and pay player B $6 if it comes up 1. If it comes up 3 or 5, he will pay $3. But if it comes 2, 4 or 6, he pays nothing. At what price would this gamble be fair?
2. a) In the game Matching Pennies, suppose player 1 thinks player 2 plays Heads with probability .7 and Tails with probability .
3. What is the expected utility to player l from playing Heads, and what is his expected utility from playing Tails? What pure strategy should player 1 choose, given these beliefs? Find beliefs player 1 could have about player 2's strategy that would rationalize player 1’s other pure strategy.
b) Think about the steps you used in part (a) to compute player 1's expected utility, given her beliefs about player 2's strategy. Why is this procedure legitimate?
c) A casino offers the following gamble: a fair coin is flipped over and over until it comes up heads. If the coin comes up heads for the first time on flip n (which happens with probability 1/2n), you win $2n and the game ends. What is the expected value of this gamble? How much would you pay to take this gamble? Explain one reason why someone might pay less than the expected value.
d) A bookie offers the following lottery: If the high temperature in Los Angeles on January 28, 2012 is at least 45o F more than the high temperature in Chicago on January 28, you win $1. Otherwise, you win $0. How much would you pay for this gamble? Argue that this is a reasonable assessment of your subjective probability estimate of the event that the high temperature in LA exceeds the high in Chicago by at least 45o F on January 28.
3. Write down the strategic form of Rock, scissors, Paper and find all Nash equilibria. (In the game, each player has three choices: Rock, Scissors, and Paper. The game is zero-sum. Rock beats Scissors, Scissors beat Paper, and 'Paper beats Rock. If both players make the same choice they tie.)
4. For each of the following simultaneous games, identify any dominant
strategies and Nash Equilibrium. In cases where both players have dominant strategies, is the outcome of playing them Pareto Efficient?
Pareto Efficient: A strategy is Pareto Efficient if there is no other strategy in which a player is better off without making other players worse off.
1 Practice:
1. Player A offers player B a gamble. For a price, he would throw a dice and pay player B $6 if it comes up 1. If it comes up 3 or 5, he will pay $3. But if it comes 2, 4 or 6, he pays nothing. At what price would this gamble be fair?
2. For each of the following simultaneous games, identify any dominant strategies and Nash Equilibrium. In cases where both players have dominant strategies, is the outcome of playing them Pareto Efficient? Pareto Efficient: A strategy is Pareto Efficient if there is no other strategy in which a player is better off without making other players worse off.
5. ("Borrowed" from Myerson, 1991; and Moulin, 1983) Chair, Ranking
Member, and Scrub are voting in a committee to choose among three options, A, B, and C. Each player submits a secret vote for one option. If any option gets two or more votes, it is the outcome. Otherwise, if there is a (three-way) tie, Chair invokes her prerogatives and chooses her most preferred option (A) as the outcome. Are there any strictly or weakly dominated strategies? Solve the game using iterated deletion of weakly dominated strategies. Would the Chair be better off if she could commit to choose an option besides her favorite in the event of a tie?
(The table is read as follows: If option A is the outcome, Chair's payoff is 8,
Ranking Member's payoff is 0, and Scrub's payoff is 4. If option B is the outcome, Chair's payoff is 4, Ranking Member's payoff is 8, and Scrub's payoff is 0, etc.)。
