流体力学与传热(英文版)chapter4(a)4.1~4.2
(流体力学与传热英文课件)Pressure drop and loss due to friction

The equation(1.4-10) is the equation usually used to calculate skin friction loss in straight pipe.
For laminar flow only, combining Eqs. (1.4-20 ) and (1.4-10) .gives
f 16 Re
64
Re
(1.4-22 )
It is not possible to predict theoretically the Fanning friction factor f for turbulent flow as was done for laminar flow.
1.4.3 Turbulent Flow in Pipes and Channels
Although the problem has not been completely solved, useful relationships are available.
• For turbulent flow the friction factor must be determined empirically, and it not only depends upon the Reynolds number but also on surface roughness of the pipe.
L R
Rearranging equation (1.4-2 ) gives
w
Rp 2L
Substituting from equation above into equation (1.4-7) gives
fV 2 w /2R V p 2//2 2 L4D L pf V 2 2
传热学英文版Chap4
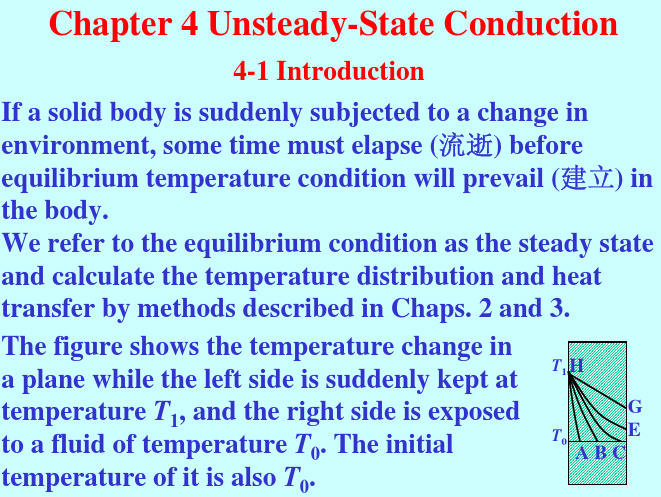
Consider a plate of thickness 2L. The plane is initially at a uniform temperature Ti and at time zero the surfaces are suddenly exposed to T = T∞. The boundary ∂T condition is: −k = h(Tw − T∞ )
θ T − T1 4 ∞ 1 −[ nπ / 2 L ] ατ nπx = = ∑ e sin θ i T − Ti π n =1 π 2L
n = 1,3,5,L
The solution for the third kind of boundary condition is:
θ ( x, τ ) = 2∑ e θi n =1
∞ 2 ατ − β ( ) L n L2
x sin( β n L) cos ( β n L) L β n L + sin( β n L) cos( β n L)
4-2 Lumped-Heat-Capacity System (集总参数系统) The analysis of transient heat conduction might be much simpler for systems which could be considered uniform in temperature. This type of analysis is called the lumped-heat capacity method. 1. Introduction
Where V/A has a dimension of length, denoted as s; and hs/k is the Biot number, BiV; ατ/s2 is called the Fourier number, (付里叶数) denoted by FoV; The subscript V means the characteristic length is V/A.
工程传热学双语 四川大学Chapter 4-convective heat transfer

4.1 Some Preliminary Concepts Regarding Convective Heat Transfer 对流传热概论 4.2 An Exact Laminar Boundary Layer Theory 边界层理论 4.3 Correlation of Heat Transfer Data 传热关联式 4.4 Heat Transfer Analysis in Pipe Flow 管内对流传热分析 4.5 Models of Convection in Laminar Flows 层流对流传热模型
流体流过壁面,而且与壁 面存在温度差时,流体与 壁面间的热量传递过程 称为对流传热。
u∞,T∞ Q
Ts
Ts < T∞ 总体要求 : • 定性—对流换热基本概念,强化对流换热的机理 • 定量—表面传热系数计算,换热量计算
对流特点 • 流体的宏观运动 + 微观的导热 • 流动与换热密不可分 • 对流换热的机理与通过紧靠换热面的薄膜层的热传导有关
thermal diffusivity 热扩散率
α = k ρC p
δ ⎛v⎞ = fn ⎜ ⎟ = fn (Pr ) Pr = v δ' ⎝α ⎠
α
fn ( Pr ) = Pr1 3
j-factor for heat transfer 传热 j 因子
f Nu = Re Pr1 3 2
jH ≡
Nu RePr 1 3
−1
friction factor f 摩擦因子
f ≡
τ
1 ρU 2 2
= CD δ ≡
1 2
μU fρ U
流体力学与传热电子教案--chapter4
Chapter 4 basic equations of fluid flowThe principles of physics most useful in the applications of the fluid mechanics are mass-balance , or continuity连续性 ; the linear- and angular-momentumbalance equation, and the mechanicalenergy balance.1Steady –state flow稳定流动if velocity , Pressure. Physical properties do not vary with time ,but can change with location ,the flow is called Steady – state flow. U=f(x,y,z)2Mass balancep56Consider the flow through a conduit管道 of cross-sectional area Sa at the entrance入口 and area Sb at the exit. The average velocity and density atthe entrance are ua and ρa; at the exit they are ub and ρbab4At steady state,the mass flow in equals the mass flow out,m& = Vρ = uaSa ρa = ubSb ρb = uSρ = const (4.11)The equation is also called the equation of continuity. 连续性方程5For an incompressible fluids不可压缩流体ρa = ρb = ρIf the fluid flows through a channels of circularcross section圆管 ,volumetric flow rate isV=uS=πud246Flow passes through two station a and b,the relationship between two velocitiesua and ub from whichV= uaπ4d2 a= ubπ4d2 bua ub=⎜⎜⎝⎛db da⎟⎟⎠⎞2(4.12)Where 7da and db are diameters直径 of the channel at the upstream and downstream stations,Macroscopic momentum balance宏观动力学平衡p66An overall momentum balance can be written for control volume shown in figure.Ma aMbb8Assuming :the flow is steady and uni-directional in the x direction.The sum of forces acting in the x direction equals the difference between the momentum leaving with the fluid per unit time and that brought in per unit time by the fluid , 流体受到的力平衡为F = (M b − M a )(4.37)9The momentum flow rate M of a fluid stream = mum --a mass flow rate u-- velocityF = m(ub − ua )velocity u--- is an average velocity at the cross section 横截面.10If u varies from point to point in the cross section of stream, however, the total momentum flow does not equal the product of mass flow rate and average velocity如果u是点速度,则上述方程不成立;11Several force components acting on the fluid in the direction of the velocity component may appear:(1) Pressure change in the direction of flow;(2) Shear stress at the boundary between the fluid stream and the conduit管道, or external forces acting on the solid wall;(3) The component of the force of gravity重力.12For one-dimensional flow, a typical situationis represented by the equationF = m(ub − ua ) = paSa − pbSb + Fw − Fg(4.43)Where pa, pb=inlet and outlet pressure, Sa, Sb=inlet and outlet cross sectionFw=net force of wall of channel on fluid Fg=component of force of gravity 13Layer flow with free surface(自学)In one form of layer flow the liquid layer has a free surface and flows under the force of gravity over an inclined or vertical surface垂直表面.If such flow is in steady state, with fully developed velocity gradients, the thickness of the layer is constant. As shown in FigureThe shear stress can be ignored at thefree liquid surface.lτIf the flow is laminar薄层 andthe liquid surface is flat平坦 andfree from ripples 波纹.rgThe fluid motion can be analyzed mathematicallyThe possible forces acting on the control volume in a direction parallel to the floware z the pressure forces at the endsz the shear forces on the upper and lower facesz the component of the force of gravity in the direction of flow.Since the pressure on the outer surface is atmospheric, the pressures on the control volume at the ends of the volume areequal and oppositely directed.Also, by assumption, the shear on the upper surface 上表面of the element单元 is neglected.The two forces remaining are therefore theshear force on the lower surface of the controlvolume and the component of the gravityin Fg cosφ −τA = 0the direction of flow.From this equation, noting thatA=bL and Fg=ρrLbgSoτ = ρrg cosφSince the flow is laminar,τ= -µdu/dr and− μ du = gμr cos φdrRearranging and integrating between limits gives∫ ∫ u du = − gρ cosφ r rdr0μδandu = ρg cosφ (δ 2 − r 2 ) 2μWhere δis the total thickness of the liquid layer•Momentum balance in potential flowBernoulli Equation 柏努力方程 p71p´spS+ΔSp+ΔpΔLConsider a volume element of a stream tube in steady potential flow, as shown in FigEq.(4.58a)is known as Bernoulli eq.without friction 无摩擦力的柏努力方程.It is a particular form of a mechanical energy balance.1m u2/2 -----kinetic energy(动能)2m gz or mgh-----Potential (位能)3p/ρ-----Pressure energy(压力能), represent the mechanical work done by forces,external to stream,on the fluid in pushing it into the tube or the work recovered from the fluid leaving the tubeDiscussion of Bernoulli equation Note:Eq.(4.58) is useful in dealing with1.For incompressible flow不可压缩流体.2. Without friction loss3. For unit mass1.Draw the picture and determine thesysytem you section.2.The section you chouse should be vertical to the flow direction .the flow between two section must be continue .the unknows you want to find are between the two section.There are four steps to solve the problem using the B.equation.3.choose the reference place (or station).4. Units of each term should be consistant. where the most information about pressure, velocity, and heights is available.应用柏努利方程如何选取截面?a.画出系统示意图,指明流体的流动方向;两个截面必须标明在图上;b.标出基准面和上、下游计算截面,两个截面必须垂直流体流动的方向;c. 被求的未知数必须包括在选取的两个截面之中;d. 两个截面之间的流体必须是连续的;e. 除了被求的未知数以外,在截面的中的其它物理量都是巳知数,或者能通过其它关系计箅出来;Bernoulli equation:correction修正for effects of solid boundariescorrection of the equation for the existence of fluid friction.problem两不相溶液体有一稳定相界面,当液体上面有一面积为S=1000cm2平板以U=0.4m/s的速度运动时,并假定速度分布为直线。
工程热力学与传热学chapter4The Second Law of Thermodynamics

The use of the second law of thermodynamics is not limited to identifying the direction of processes, however. The second law also asserts that energy has quality as well as quantity. The first law is concerned with the quantity of energy and the transformations of energy from one form to another with no regard to its quality. Preserving the quality of energy is a major concern to engineers, and the second law provides the necessary means to determine the quality as well as the degree of degradation of energy during a process. As discussed later in this chapter, more of high-temperature energy can be converted to work, and thus it has a higher quality than the same amount of energy at a lower temperature.
4-1 Irreversible process
热功转换模 拟图
1)热—功转换的方向性
4-1 Irreversible process
流体力学与传热(英文版)Chapter4(d)4.5

t tLstw Nhomakorabeas
D
t
w
(a)
(b)
(c )
• (a) and (b) film type, (c) dropwise
In film condensation
the liquid condensate forms a film, or continuous layer, of liquid that flows over the surface of the tube under the action of gravity.
• Friction losses in a condenser are normally small, so that condensation is essentially a constant-pressure process.
• The condensing temperature of a single pure substance depends only on the pressure, and therefore the process of condensation of a pure substance is isothermal.
4.5.1.1 Dropwise and film-type condensation A vapor may condense on a cold surface in one of two ways, which are well described by the terms dropwise and film-type.
Phase change covers condensation, boiling, melting, crystallization and sublimation (升华, 凝华)
流体力学与传热课件Centrifugal pump theory

2
2
r2
2
g
ΔH is the ideal theoretical head developed
In practice, the total head across the pump is less than this due to energy dissipation in eddies and in friction.
The capacity Q is proportional to the diameter D, the head H is proportional to D2, and the brake horsepower W is proportional to D3.
The developed head of an actual pump is considerably less than that calculated from the ideal pump relation.
Pump performance also depends on β2.
β2 > 90o are forward facing and Head
The velocity V has radial and
tangential components Vr and Vt, respectively. The angular momentum of a mass m of
Chapter_44(图)-总结

A SUMMARY forChapter 4: Convective Heat Transfer4.0 关于雷若数 Re 、努谢尔数Nu 、普朗特数Pr 、传热因子H j 、斯坦敦数St定义式: μρDu =Re ,k hD =Nu ,p C k ρρμαν//Pr ==,3/1H Pr Re Nu =j ,Pr Re Nu St =其中,k 、、μρ、p C 为流体基本物性参数,αν、为组合物性参数;u 为定性速度,一般取流体平均速度,是流体流动参数;h 是换热系数,对应于局部(或平均)h ,称Nu 为局部(或平均)Nu 数; D 是定型尺寸,通常:• 对于在圆管内的换热(内部流动):D 为管内直径D • 对于沿平板表面的换热(外部流动):D 为平板长度L• 对于绕圆管/柱/球的换热(外部流动):D 为圆管/柱/球的外直径D4.1 重点讲述传热分析近似理论—膜理论——传热准数Nu 的间接计算方法假设:流体在沿壁面流动换热的过程中,流体的速度和温度变化分别限制在壁面附近的一个很薄的流体层内,分别称为动量边界层和热边界层。
动量边界层内流体的速度为线性分布,热边界层内流体的温度也为线性分布,由此进行分析得到一个重要结果,即所谓的Colburn 类比:2Pr Re Nu 31fj H =≡(4.1.19) 该式表明: Nu 数可间接通过流体流动摩擦系数f 来求取。
例如: 对于圆管内的湍流换热∵25.0Re 079.0-≈f ∴33.00.75Pr 0.04Re Nu = (实验33.00.8Pr 0.023Re Nu =) 对于沿平板的层流换热(Re 和Nu 中的定性尺寸为平板长度L )∵5.0Re 328.1-≈f ∴33.00.5Pr 0.664Re Nu = (实验33.00.5Pr 0.68Re Nu =)4.3 重点讲述常用的传热准数Nu 实验关联式——传热准数Nu 的直接接计算方法 4.3.1 圆管内湍流流动换热 (层流流动换热关系式见4.5节) Friendz-Metzner 关联式()()3121p Pr 1Pr 28.112.12RePr Nu St --+==≡f f G C h (4.3.3) 其中 32.0Re 125.00014.0+=f (4.3.4)Dittus-Boelter 关联式nPr 0.023Re Nu 0.8= (流体被加热n =0.4, 被冷却n =0.3)4.3.2 横掠圆管(柱)外流动换热52.03.0Re 56.035.0Pr Nu += (4.3.5) 4.3.3 绕球形颗粒流动换热流体为空气时,由雷若数Re 查图4.3.2得传热准数Nu 。
工程热力学与传热学(英文) 第4章 热力学第二定律

1
T
q1
2
T1 q= 0 T2
3
1
2
q= 0
4
4
3
q2
0
v
0
Δs
s
3. The Efficiency of a Carnot Engine(卡诺热效率)
For any heat engine wnet q 1q 2 q2 t 1 q1 q1 q1 For a Carnot engine Method 1: From T-s diagram
A
the paddle wheel • The internal energy of the gas is increased
• The reverse process, raising the
B
mass by transferring heat from the fluid to the paddle wheel, does not occur in nature.
2. p-v and T-s Diagrams
p
1
T q1
2
T1
1
2
q= 0
4
q= 0
q2
3
T2 v 0
4
3
0
Δs
s
• 1-2 Reversible isothermal expansion
(T1=constant, heat absorbed q1) • 2-3 Reversible adiabatic expansion • 3-4 Reversible isothermal compression (T2=constant, heat rejected q2) • 4-1 Reversible adiabatic compression
流体力学与传热(英文版)Chapter4(f)4.7

4.7 heat-exchange equipment
In industrial processes heat energy is transferred by a variety of methods. Heat exchangers are of importance for heat energy transport. There are lots of exchangers. Including conduction-convection in exchangers, boilers, and condensers; radiation in furnaces and radiant heat dryer.
Multipass construction increases the fluid velocity, with a corresponding increase in the heat-transfer coefficient.
An even number of tube-side passes are used in multipass exchangers. The shell side may be either single-pass or multipass.
Flash p343
The disadvantages of multipass are that
(1) the exchanger is slightly more complicated ; (2) Some sections in the exchanger have parallel flow, which limits the temperature approach; (3) the friction loss rough the equipment is increased because of the larger velocities and multiplication (增大) of exit and entrance losses.
流体力学(英文版)

流体力学(英文版)Fluid MechanicsCourse Code: 83165000Course Name: Fluid MechanicsCourse Credit: 3Course Duration: The 3rd SemesterTeaching Object: Undergraduate Students in Space Science Pre-course:Advanced Mathematics, General PhysicsCourse Director: Shi Quanqi Lecturer Philosophical Doctor Course Introduction:Fluid Mechanics is a foundation course which is used extensively in the engineering .At the same time, it is also a basic course in learning magnetohydrodynamics (MHD)。
The course includes the concept of the continuum, kinematics, the hydrodynamics Equations, the similarity theory and dimensional analysis, and the dynamics of gas flow. Application examples in fields of power source, dynamics, Aviation, machinery, space science and daily life will be introduced. When the students finish this course, they will be able to understand the basic concept, the general theory and the analytical method in Fluid Mechanics, and will know its application in the Space Sciences, engineering, and our daily life.Course Examination:Students’ Final Scores = Scores of Ordinary Tests * 30% + Scores of the Final Exam * 70%;Scores of ordinary tests vary according to students’ performance in class and homework.;The final exam will be open-book.Appointed Teaching Materials:[1] Ding Zurong,Fluid Mechanics,Beijing:China HigherEducation Press,2005.Bibliography:[1]. Wu Wangyi,Fluid Mechanics,Beijing:Peking University Press,2004.。
流体力学(英文课件)chap5

分 量 形 式
u x u x u x u x 1 p ux uy uz X t x y z x
4 equations for 4 quantities
u y
ux
u y
uy
u y
uz
u y
1 p y 1 p Z z u z 0 z Y
16
4. Incompressible 2. The Navier-Stokes Equations (3) Ideal Fluid Flow
p xx dx)dydz zx dxdy ( zx zx dz)dxdy x z yx du yx dxdz ( yx dy)dxdz dxdydz x y dt
E
z
F p yy
yz
xz p xx
A G
H
1. index: normal vector on face 2. index: direction of force
xy
D
yx
B
O x 图3-21
zx p zz
y
zy
C
p xx xy xz yx p yy yz p zz zx zy
1 p az Z z
Fluid Mechanics 2010 CN Chapter 4
10
4 - 1 理 想 流 体 的 运 动 方 程
欧拉运动微分方程
1、采用六面体微元分析法
2、加速度中含当地加速度和迁移加速度
3、x轴方向:
p dx p dx p dydz p dydz X dxdydz dxdydzax x 2 x 2
流体力学与传热课件Heat Transfer and Its Applications

On the other hand, k is a function of temperature, but not a strong one.
The negative sign reflects the physical fact that heat flow occurs from hot to cold and the sign of the gradient is opposite that of the heat flow.
In using equation it must be clearly understood that the area A is that of a surface perpendicular to the flow of heat and distance n is the length of path measured perpendicularly to area A.
• k vary over a wide range. They are highest for metals and lowest for finely powdered materials from which air has been evacuated.
Fourie’s law states that k is independent of the temperature gradient.
4.2 Heat Transfer by Conduction
Conduction is most easily understood by considering heat flow in homogeneous isotropic solids because in these there is no convection and the effect of radiation is negligible.
流体力学与传热习题参考解答(英文)

1. Water is pumped at a constant velocity 1m/s from large reservoir resting on the floor to the open top of an absorption tower. The point of discharge is 4 meter above the floor, and the friction losses from the reservoir to the tower amount to 30 J/kg. At what height in the reservoir must the water level be kept if the pump can develop only60 J/kg?2222112f 1U P U P w=Z g+h (Z g+)22ρρ++-+ U 1=0 12P =P 10Z = W=60j/kg f h 30/kg =2U =1m/s 2(60300.5)/g 3m Z =--=21Z Z Z 431m ∆=-=-=2. The fluid (density 1200 kg/m 3 ) is pumped at a constant rate 20 m 3 /h from the large reservoir to the evaporator. The pressure above the reservoir maintains atmosphere pressure and the pressure of the evaporator keeps 200 mmHg (vacuum). The distance between the level of liquid in the reservoir and the exit of evaporator is 15 meter and frictional loss in the pipe is 120 J/kg not including the exit of evaporator, what is the pump effective work and power if the diameter of pipe is 60 mm?22112212f U U Z g+W Z g+h 22ρρP P ++=++ 10P = 5422200P x1.013x10 2.67x10N /m 760=-=- 31200Kg /m ρ= 1U 0= f h 120J /kg =22V 20U 1.97m /s A 3600*4006===π/*. 1Z 0= 2Z 15= 422.67x101.97W 15x9.81120246.88J /kg 12002=-+++= N W Q 246.88x1200x20/3600=1646W ρ==3. Water comes out of the pipe (Φ108x4 mm), as shown in Fig. The friction loss of the pipeline which does not cover the loss at the exit of pipe can be calculated by the following equation:h f =6.5U 2where U is the velocity in the pipe, finda. water velocity at section A-A'.b. water flow rate, in m 3 /h. 22112212f U U Z g+Z g+h 22ρρP P +=++ 1U 0= 12P =P 1Z 6m = 2Z 0=2f h 6.5U = 22U 6x9.81 6.5U 2=+ U 2.9m/s = 23V=UA=2.94x01x360082m /h =π/.4. Water passes through the variable pipe. The velocity in the small pipe is 2.5 m/s. The vertical glass tubes are inserted respectively at the section A and B to measure the pressure (see fig.) If the friction loss between two section is 15 J/kg, what is the water column difference between two glass tubes? By the way, draw the relative liquid column height of two tubes in the Fig.a ab b U A U A = 2b U 2.5*(33/47)1.23m /s == 22a a b b a b f U U Z g+Z g+h 22ρρP P +=++ a b Z =Z 22a b b a f U U h 22ρρP P -=-+221.23/2 2.5/21512.63=-+= a b P P R g ρ-=∆ 3312.63R=1.29x10m 9.8x10-∆=5. A centrifugal pump takes brine (density 1180 kg/m 3 , viscosity 1.2 cp) from the bottom of a supply tankand delivers it into another tank. The line between the tanks is 300 m of 25 mm diameter pipe (inner diameter). The flow rate is 2 m 3 /h. In this line, there are two gate valves, four elbows (90o ) and one return bend, what is the friction loss if the roughness of pipe is 0.025 mm?22f fst flocal U U h h h 4f k d 22l ∑=+=+∑ 31180kg /m ρ= 300m, d=0.025m l =3-3v 2m /h =1.2cp=1.2x10Pa.s μ=k=0.025mm k/d=0.025/25=0.001c l r k =0.4 k =1 k =2x0.07=0.14el re k 4x0.75 3 k 1.5-2.2===2u v /A 2/(3600x /4x0.025)1.13m /s π===4u d Re 2.78x10ρμ== f 0.063= 2f 2h 4x0.0063x300/0.025x1.13/2+(0.4+1+2x0.07+4x0.7+1.5)x1.13/2 =197.86J/kg∑=6. The orifice meter (diameter of orifice 0.0001 m) is installed for measuring the flow rate. The indicating liquid of orifice is mercury if U shape pressure gauge reading is 0.6 meter and orifice coefficient can be takenas 0.61, what is the flow rate of water?o u c =20o 0V u s 0.61x /4x0.0001π==835.8x10m /s -=7. Water flows through a pipe with a diameter di 100 mm as shown in figure.a. when the valve is closed, R is 600 mm and h equals 1500 mm. While the valve opens partially, R=400 mm and h=1400 mm, f=0.00625 (Finning factor) and k c =0.5 (contraction coefficient), what is the flow rate of water, in m 3 /h?b. If the valve opens fully, what is the pressure of section 2-2', in N/m 2 ? The equivalent length of the valve is1.5 m and the Fanning factor f keeps the same?(ρH2O =1000kg/m 3, ρHg =13600kg/m 3)(1) the valve opens partially ,for selection 1-1’ and 2-2’ , we have 22112212f 1-2u u gZ gZ h 22ρρP P ++=+++ 2212Hg H o 0 g(R h)39630N/m ρρP =P =-= 2212f1-2c u u u 0 Z =0 h 4f +k 2.13u d 22===l We can get Z1 from the valve closed21Hg H O h=1.5m R=0.6m Z gR/h 6.66m ρρ=-=229.81x6.66u /2 2.13u 39630/1000=++23h u=3.13m/s V 3600x /4x0.1x3.1388.5m /h π==(2) when the valve opens fully, for section 1-1’ and 3-3’, we have 22331113f1-3u u gZ gZ h 22ρρP P ++=+++ 311Z 0 Z 6.66m u =0== 22e f1-3c u 3.1.5h (4f k )(4x0.00625x +0.5) 4.81u d 20.01l l ++=+== 229.81x6.66u /2 4.81u =+ u 3.51m/s =For section 1-1’ and 2-2’22112212f1-2u u gZ gZ h 22ρρP P ++=+++ 112120 Z 6.66 Z 0 u 0 u 3.51P ===== 22f1-2c l u h (4f k )(4x0.00625x15/0.10.5)3.51/226.2J /kg d 2=+=+= 22229.81x6.66 3.15/226.2N 32970mρP =++P =8. The rotameter is installed to measure the water flow rate, as shown in figure. If the total length including equivalent length of pipeline A is 10 m and the reading of rotameter is 2.72 m 3 /h, what is the flow rate for pipeline B? (f A =0.0075, f B =0.0045)For parallel pipe line fA fB total A B22A fA A 2A h h V V +V u (l+le) 2.72h 4f 4x0.0075x10/0.053/2()d 23600x /4x0.053π∑=∑=∑∑== 0.333J /kg = 22B fB B B B 23B B B B u (l+le)h 4f 4x0.0045x2/0.3/2xu 0.333d 2u 2.36m /s V =u A 2.36x /4x0.23600m /h π∑∑======10. A flat furnace wall is constructed of 120 mm layer of sil-o-cel brick, with a thermal conductivity 0.08 w/(m o C), backed by a 150 mm of common brick, of conductivity 0.8 w/(m o C), the temperature of inner face of the wall is 1400 o , and that of the outer face is 200o C.a. What is the heat loss through the wall in w per square meter.b. To reduce the heat loss to 600 w/m 2 by adding a layer of cork with k 0.2 w/(m o C) on the outside of common brick, how many meters of cork are requied? a. 2Q t 1400200711N /m 11L R 0.080.80.120.15∑∆-===∑+ b. 600=(1400-200)/(0.12/0.08+0.15/0.8+x/0.2)x=0.0625m13. Air at the normal pressure passes through the pipe (d i 20 mm) and is heated from 20o C to 100o C. What is the film heat transfer coefficient between the air and pipe wall if the average velocity of air is 10 m/s? The properties of air at 60 o C are as follows:density 1.06 kg/m 3 , viscosity 0.02 cp, conductivity 0.0289 w/(m o C), and heat capacity 1 kJ/kg-K443u d 10x0.02x1.06 Re=1.06x10100.02x10ρμ-==>12T +T 20100T=6022+==℃ 0.141ωμμ⎛⎫= ⎪⎝⎭10000.020.0010.6920.0289p c x x k μ==Pr= ()()0.81/3081/34Nu 0027Re Pr 0.027x 1.06x10x 0.69239.66==.=. ()2i i i h d 39.66 h 39.66x0.0289/0.02=57.22w/m .k k ==14. A hot fluid with a mass flow rate 2250 kg/h passes through a ∅25x2.5 mm tube. The physical properties of fluid are as follows:k=0.5 w/(m o C), C p =4 kJ/kg-K, viscosity 10-3 N-s/m 2 , density 1000 kg/m 3 Find:a. Heat transfer film coefficient h i , in w/(m 2 -K).b. If the flow rate decreases to 1125 kg/h and other conditions are the same, what is the h i ?c. If the diameter of tube (inside diameter) decreases to 10 mm, and the velocity u keeps the same as that of case a, calculate h i .d. When the average temperature of fluid and quantity of heat flow per meter of tube are 40 o C and 400 w/m, respectively, what is the average temperature of pipe wall for case a?e. From this problem, in order to increase the heat transfer film coefficient and enhance heat transfer, what kinds of methods can you use and which is better, explain?Hint: for laminar flow, Nu=1.86[Re Pr]1/3for turbulent flow Nu=0.023Re 0.8 Pr 1/3 (1) 444N 2250x4u d Gd d 3600x x0.02Re 3.98x10100.001ρππμμμ=====> ()()1/30.8081/3424Nu 0023Re Pr 0.023x 3.98x10220.10.5Nuk 220.1x0.5hi 5500w /m k d 0.02⎛⎫== ⎪⎝⎭===.=. (2) 12w 2w = 4421Re Re /2=2x1010=> 0.80.82211Nu Re 0.5Nu Re ⎛⎫== ⎪⎝⎭ 0.8i2i1h 0.5h = ()0.82i2h 5500x0.53159w /m k == (3) 44333u d 2000x0.01Re 2x10100.001ρμ===> 0.81/3Nu 0.023Re Pr = ()2hi=6347w/m k(4)i i w w Q=h A (t-t )=400=500x2x0.02(t-t )πw t=40t 39.41=℃ ℃(5) there methods : increase u or hi or decrease dThe first is better15. In a double pipe exchange (Φ23x2 mm), the cold fluid (Cp=1 kJ/kg, flow rate 500 kg/h) passes throughthe pipe and the hot fluid goes through the outside. The inlet and outlet temperatures of cold fluid are 20 and 80 o , and the inlet and outlet temperatures of hot fluid are 150 and 90o , respectively. The h i (film coefficient inside pipe) is 700 w/(m 2 o C)and overall heat transfer coefficient U o (based on the outside surface of pipe) is 300w/(m 2 o C), respectively. If the heat loss is ignored and the conductivity of pipe wall (steel) is taken as 45 w/(m o C), find:(1) heat transfer film coefficient outside the pipe h o ?(2) the pipe length required for counter flow, in m?(3) what is the pipe length required if the heating medium changes to saturated vapor(140 o C) and it condenses to saturated liquid and other conditions keep unchanged?(4) When the exchanger is used for a year, it is found that it cannot meet the need of production (the outlet temperature of cold fluid cannot reach 80 o C), explain why? (a) 0m o 0i i m d l d 111230.002x23h Vo h d kd 300700x1945x21⎛⎫=-+=-- ⎪⎝⎭ 1/h0=1/U0-(do/hidi+bdo/kdm)=1/300-23/700*19-0.002*23/45*21()20h 642.9w/m k =12t +t LMTD=702∆∆℃= Q=UoAo ∆Tm=mcCp(Tcb-Tca) 300*2π*0.023*70L=500/3600*1000*(80-20)L=5.4m(c) 8020LMTD=86.514020ln 14080-=--℃ 1122L t 70/86.5L t ∆==∆ 2L 0.81L1 4.4m == (d) scale is formed on the outside ,V 0 is decreased16. Water flows turbulently in the pipe of Φ25x2.5 mm shell tube exchanger. When the velocity of water u is 1 m/s, overall heat transfer coefficient Uo (based on the outer surface area of pipe) is 2115 w/(m 2 o C). If the u becomes 1.5 m/s and other conditions keep unchanged, Uo is 2660 w/( m 2 o C ). What is the film coefficient ho outside the pipe? (Heat resistances of pipe wall and scale are ignored)o i h h Uo 111+= (1) oi o h h U 1'1'1+= (2) (1)-(2)= 0.80.80.80.81211111121152660u C u C 1C 1.5C-=-=- C=2859 io h Uo h 111-= ho=8127W/(m2K)17. Water and oil pass parallelly through an exchanger which is 1 m long. The inlet and outlet temperatures of water are 15 and 40 o C, and those of oil are 150 and 100 o C, respectively. If the outlet temperature of oil decreases to 80 o C, and the flow rates and physical properties and inlet temperatures of water and oil maintain the same, what is the pipe length of new exchanger? (Heat loss and pipe wall resistance are neglected) ()()h h 12c c 21m Q W C T -T W C t t VA t ==-=∆()()h h 12c c 21W C T -T 'W C t 't =-2150100401515080t 15--=-- 2t 50=℃ 212m1112m2L T T 't 1508092.51.85L T T t 15010069.8-∆-===-∆- 2m1m2L 1.85m L1=1m t 92.5 t 69.8=∆=∆=18. Air which passes through the pipe in turbulent flow is heated from 20 to 80 o C. The saturated vapor at 116.3 o C condenses to saturated water outside the pipe. If air flow rate increases to 120% of the origin and inlet and outlet temperatures of air stay constant, what kind of method can you employ in order to do that? (Heat resistance of pipe wall and scale can be ignored))(111ca cb pc c m i i T T C m T A h -=∆=1Q)'(2212ca cb pc c m i T T C m T A h -=∆=2Q 128.012112i22.12.1h m m c c m i m T T m m T h T ∆∆===∆∆ )803.116/()203.116ln(20801---=∆m T )80/()20ln(20802---=∆h h m T T T Th=118.5oC19. Water flows through the pipe of a Φ25x2.5 mm shell-tube exchanger from 20 to 50 o C. The hot fluid (C p1.9 kJ/kg o C, flow rate 1.25 kg/s) goes along the shell and the temperatures change from 80 to 30 o C. Film coefficients of water and hot fluid are 0.85kw/(m 2 o C) and 1.7 kw/(m 2 o C). What is the overall heat transfer coefficient Uo and heat transfer area if the scale resistance can be ignored? (the conductivity of steel is 45w/(m o C).W=1.25Kg/s Cp=1.9Kj/kg ℃()()2h p 12Q W C T T 1.25x1.9x 80-30119Kw =-==m 3010t 30ln 10-∆= ()200m 00i i m 1V 472w/m k d l d 1h h d kd ++==32i 0m Q 119x10A 13.9m V t 472x18.2===∆20. A spherical particle (density 2650 kg/m 3) settles freely in air at 20 o C (density of air 1.205 kg/m 3 , viscosity 1x10-5 Pa.s). Calculate the maximum diameter of particle if the settle obeys the Stoke s’ Law?Re ≤1 ()2p t p D g U 18D ρρμμρP -== ()23p 18D g μρρρP =- ()1/3-10p 18x10D 1.205x9.81x 2650-1.205⎛⎫= ⎪ ⎪⎝⎭=3.85x10-521. A filter press(A=0.1 m 2 ) is used for filtering slurry. The vacuum inside the filter is 500 mm Hg. One liter filtrate can be got after filtering of 5 min and 0.6 more liter filtrate is obtained after 5 more min. How much filtrate will be got after filtering of 5 more min?for filter press 22e V 2VV =KA θ+5 min 22e 12V 0.1x5K +=(1)10min 22e 1.62x1.6V 0.1x10K +=(2)From (1) (2),we can see Ve=0.7 K=4815 min 22V 2x0.7V=48x0.1x15+ V=2.07m 3/h22. The following data are obtained for a filter press (A=0.0093 m 2) in a lab.------------------------------------------------------------------------------------------------pressure difference (kg f /cm 2 ) filtering time (s) filtrate volume (m 3 )1.05 502.27⨯10-3660 9.10⨯10-33.50 17.1 2.27⨯10-3233 9.10⨯10-3Find1) filtering constant K, q e , t e at pressure difference 1.05 kg f /cm 2 ?2) if the frame of filter is filled with the cake at 660 s, what is the end filtering rate (dV/dt)E at P 1.05 kg f /cm 2 ?3) compressible constant of cake s?For p=1.05Kg/cm 22e 2e 2e q 2qq K 0.002270.0002272x q 50K 0.00930.000930.000910.000912x q 660K 0.000930.00093θ+=⎛⎫+= ⎪⎝⎭⎛⎫+= ⎪⎝⎭We can see K=0.015 qe=0.026For p=3.5Kg/cm 21-s K=2k ∆P 1-s K'=2k '∆P 1s K 'K '-∆P ⎛⎫= ⎪∆P ⎝⎭ ()2E e V KA 2V+V d d θ⎛⎫= ⎪⎝⎭23. A slurry is filtered by a 0.1 m 2 filter press at constant pressure if the cake is incompressible. The filter basic equation is as follows:(q+10)2 = 250(t+ 0.4)where q---l/m 2 t----minfind (1) how much filtrate is got after 249.6 min?(2) if the pressure difference is double and the resistance of cake is constant, how much filtrate can be obtained after 249.6 min? (cake is imcompressible)(1)let θ=249.6 ()()2q+10250x 249.60.4=+ q=240 V=qA=240*0.1=24(2) K 2k =∆P K'2k '=∆P'2∆P =∆P K'2K 500== ()()2q'+10500x 249.60.4=+ q ’=343.6 v=34.36。
流体力学与传热(英文版)Review

Velocity distribution of laminar flow
r ur 1 umax rw
Hale Waihona Puke 2Where umax=-pri2/4L
ur is a local velocity
Laminar Flow
If the flow is laminar, the following is satisfied. 1 parabolic profile of velcity
The
relationship between gauge pressure and absolute pressure as well as vacuum
Fluid-flow phenomena
Ideal
fluid
Boundary layer
Two
kinds of flow patterns
Laminar flow Turbulent flow Criterion of flow pattern:
Re d u
Newtonian fluid
The shear stress is proportional to the shear rate (Newtonian Law of viscosity)
Unit
Example:
what is the dimension and unit of viscosity, in SI system?
Unit
conversion: P=1atm=?mmHg=?mH2O=?N/m2
Fluid
Statics and Its Applications
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Note that the linear dependence on the temperature driving force ts-tf is the same as that for pure conduction in a solid of constant thermal conductivity.
Chapter 4 Heat Transfer and Its Applications
4.1 Introduction
It’s hot summer, you are going to have a lunch, a bowl of noodles.
Nature of heat flow
When two objects at different temperatures are brought into thermal contact , heat flows from the object at the higher temperature to that at the lower temperature. The net flow is always in the direction of the temperature decrease.
q h(ts t f ) A
(4.1-2)
Where: q: heat transfer rate, w A: heat transfer area, m2 ts : surface (wall) temperature, K tf: fluid temperature, K h: heat-transfer coefficient , w/(m2 .K ) h is not a physical property of the fluid, but depends on the flow patterns determined by fluid mechanics as well as on the thermal properties of the fluid.
Convection
When a current or macroscopic particle of fluid crosses a specific surface, such as the boundary of a control volume, it carries with it a definite quantity of enthalpy. Such a flow of enthalpy is called a convective flow of heat.
dq dt k dA dx
(4.1-1)
Where: q: heat transfer rate, w=J/s A: heat transfer area, m2
dq/dA:
heat transfer flux, w/m2 t: temperature, K or oC x: distance, m dt/dx: temperature gradient, K/m k: thermal conductivity, w/(m.K), it is physical property.
In metals:
thermal conduction results from the motion of free electrons.
In solids :
poor conductor of electricity, produced by momentum transport .
In most liquids: thermal conduction results from momentum transfer between adjacent vibrating molecules or atoms. In gases: conduction occurs by the random motion of molecules.
The mechanisms by which the heat flows are:
Conduction Convection Radiation
Conduction
If a temperature gradient exists in a continuous substance, heat can flow unaccompanied by any observable motion of matter.
Math. Model by Conduction
According to Fourier’s law, the heat flux is proportional to the temperature gradient and opposite to it in sign. For onedimensional heat flow
Convection
Double pipe heat exchanger(Fig.4.6)
Shell-tube heat exchanger
Math. Model by convection
The convection flux is usually proportional to the difference between the surface temperature and temperature ton’s law of cooling
Two Kinds of Convections
First one is natural (free) convection
Definition: If the currents are the result of buoyancy forces generated by differences in density and the differences in density are in turn caused by temperature gradients in the fluid mass, the action is called natural convection.
