2015年美国“数学大联盟杯赛”(中国赛区)初赛六年级试卷

六年级美国大联盟计算专题讲义(含题目翻译答案解析)

六年级美国大联盟第一阶段-计算专题（教师版）学生/课程年级学科授课教师日期时段核心内容美国大联盟——计算专题课型null教学目标null重、难点null导学一知识点讲解 1、四舍五入专业词汇总结：tenth：十分位 hundredth：百分位 thousandth：千分位ten 十 hundred 百千 thousand million 百万 billion 十亿round to：四舍五入到round to the nearest tenth 四舍五入到十分位round to the nearest ten 四舍五入到十位例题1. [单选题] [近似数及其求法] [难度：★★★ ] （2016 年第 2 题）To the nearest ten, 54.545=.A .50 B. 54.5 C. 54.6 D. 60【参考答案】A【题目解析】54.545保留到整十数是.2. [ 单选题 ] [ 近似数及其求法 ] [ 难度：★★★ ] (2015 年第 23 题）The number N when rounded to the nearest hundredth is 20.16. When rounded to the nearest thousandth, N is at mostA.20.159B.20.160C.20.164D.20.165【参考答案】C【题目解析】数字 N 四舍五入到百分位是20.16，当它四舍五入到千分位最大可能是我爱展示1. [单选题] [近似数及其求法] [难度：★★★ ] （2011 年第 3 题）What is 0.3456 rounded to the nearest hundredth?A.0.34B.0.35C.0.345D.0.346【参考答案】B【题目解析】0.3456四舍五入到百分位是多少？2. [单选题] [近似数及其求法] [难度：★★★ ] （2011 年第 10 题）(8.426 rounded to the nearest tenth )× （7531 rounded to the nearest hundred ）=A.6300B.63450C.63750D.64005【参考答案】A【题目解析】8.426四舍五入到十分位乘7531四舍五入到百位等于多少知识点讲解 2、分数运算专业词汇总结：reciprocal：倒数 fraction:分数 a unit fraction: 分数单位 numerator：分子denominator：分母whole number：整数eg: This must be a whole number between-120 and127. integer number:整数eg: I demand that you give me a positive integer.例题1. [单选题] [分数计算] [难度：★★★ ]A. B. C. D.【参考答案】B2. [单选题] [分数计算] [难度：★★★ ] （2016 年第18 题）The reciprocal ofplus the square of minus the cube of isA. B. C. D.【参考答案】C【题目解析】的倒数加上的平方减去的立方是3. [单选题] [分数计算] [难度：★★★ ] （2015 年第 6 题）How many numbers have their reciprocals equal to themselves?A.0B.1C.2D.3【参考答案】B【题目解析】有多少个数字的倒数等于它本身4. [单选题] [分数计算] [难度：★★★ ] （2011 年第12 题）(The reciprocal of)÷(the reciprocal of )=A.1B. C. D.【参考答案】B【题目解析】的倒数除以的倒数等于？5.[单选题] [分数计算] [难度：★★★ ] （2016 年第 29 题）A unit fraction is a fraction whose numerator is 1 and whose denominator is whole number. The number of unit fractions between and isA.18B.19C.20D.21【参考答案】A【题目解析】大小在到之间的，分子是1且分母是整数的分数有多少个？6.[分数计算] [难度：★★★★ ] （2011 年第 40 题）Find the fraction number with the smallest possible integer numerator that is between andAnswer:【参考答案】【题目解析】找出在和之间最小整数分子的分数我爱展示1. [单选题] [分数计算] [难度：★★★ ]A. B. C. D.【参考答案】B2. [单选题] [分数计算] [难度：★★★ ] （2015年第15题）B.4 D.8A. C.【参考答案】A3. [单选题] [分数计算] [难度：★★★ ] （2014 年第 15 题）A.2B.3C.D.【参考答案】A4. [单选题] [分数计算] [难度：★★★ ] （2012 年第 9 题）A. B. C. D.【参考答案】C5. [单选题] [分数计算] [难度：★★★ ] （2014 年第 6 题）Multiplying a number by is the same a s dividing it by ( )A.0.667B.0.75C.1.5D.1.667【参考答案】C【题目解析】一个数乘相当于这个数除以（）.6. [单选题] [分数计算] [难度：★★★ ] （2014 年第 34 题）将 0.75 转换为分数，这些分数的分子和分母都是＜100 的正整数。

.六年级.a卷.全国初赛试卷(学生版)演示教学

2015.六年级.A卷.全国初赛试卷(学生版)世界少年奥林匹克数学竞赛 2015年(全国初赛)总决赛 (本试卷满分140分,考试时间120分钟)考生须知：1.每位考生将获得试题卷及答题卷各一份,考试期间不得使用计算器及手机；2.答案写在答题卷上,写在试题卷上无效.考试完毕,试题卷及草稿纸将被回收.六年级试题(A 卷)一、选择题(共5题,每题4分,共20分)以下每题的四个选项中,仅有一个是正确的.请将表示正确答案的英文字母写在答题卷上1. 比较下面五组答案中,哪个结果最大：A .∆∆1- B .∆∆2- C .1-2-∆∆ D .23-+∆∆2. 7个18连乘,乘积等于________． A ．612220002 B ．612220012C ．612220022D ．6122200323. 邋遢大王的袜子筐中有12只脏袜子和20只干净袜子．这天他刚起床,就迷迷糊糊地从筐中拿袜子,每次拿两只,如果其中有脏袜子就会把两只都扔到地上,然后从筐中重新拿．那么他至少拿______次,才能保证一定拿到两只干净袜子． A ．7 B ．9 C ．11D ．134. 某国家的纸币有1元、5元、10元、20元、50元、100元这6种,为了能恰好支付1元至100元这100种整数元钱数,最少需要准备纸币______张． A ．8 B ．9 C ．10D ．115. 4个孩子站在8个有不同编号的方格中（如图所示）,每格最多站一人,使得每列恰有1个孩子,且每行的总人数都是奇数,有_____种符合要求的站法． A ．2B ．4C ．8D ．16C A BD GE F H二、判断题(共5题,每题4分,共20分)下列题目中的说法有的正确,有的错误,请你为每道题目判断.对的在括号里画√,错的在括号里画×.1. 一件衣服先提高15,再降低15,它的价格没有变.…………………………（）2. 一个小于200的数,它除以11余8,除以13余10,这个数是140…………… （）3. 分子分母都由两位质数构成,且分数值在15和16之间的分数有7个. ……………（）4. 在数列1,4,8,10,16,19,21,25,30,43中,相邻若干数之和,能被11整除的数组有6组.……………………………………………………………………………（）5. 一个自然数n ,已知3n 的末尾三位是888,则n 的最小值是192. …………………（）三、填空题(共20题,每题5分,共100分)1.2. 某运输队运一批大米,第一天运走总数的51多60袋,第二天运走总数的41少60袋,还剩下220袋没有运走,这批大米原来一共有________袋.3. 小明带着一些钱去买钢笔,如果钢笔降价10%,则可以比原来多买30支.那么降价10%后,小明带的钱可以买________支钢笔.4.如图,两个正方形并排靠着,边长分别是12厘米、8厘米,BD中点为H,则三角形BFH的面积是________平方厘米.HG FEDBA5.四个分数998661、99986661、116103和22602130最大的是:__________.6.如图：以三角形ABC的AB边为轴旋转一周得到一个几何体,则这个几何体的体积是___________（取3）.7.如图,用红、橙、黄、绿、青、蓝、紫7种颜色在方格纸上自左上到右下的斜行里按顺序涂色,则第20行（从上往下）的第30个格子（从左往右）涂的颜色是_________颜色.8.求20155除以9的余数_________.9.求下图阴影部分的面积____________（单位：厘米）.红橙黄绿···橙黄绿黄绿绿···10.冲冲老师和程程老师各有一个20人班,冲冲老师班上的男生平均分、女生平均分分别为86分、90分,程程老师班上的男生平均分、女生平均分分别为85分、89分；但是,冲冲老师班上总的平均分比程程老师班上总的平均分低1分,问：冲冲老师班上男生比程程老师班上男生多_______人.11.如果一个自然数的各个数码之积加上各个数码之和,正好等于这个自然数,我们就称这个自然数为“少年数”.例如,99就是一个巧数,因为9×9+(9+9)＝99.可以证明,所有的少年数都是两位数,则两位少年数共有___________个.12.一个数的完全平方数有39个因数,求该数的因数个数是__________.13.已知长方形ABCD的面积为70厘米,E是AD的中点,F、G是BC边上的三等分点,求阴影EHO△的面积是_________厘米.ADH OCB14.一个快钟每时比标准时间快1分,一个慢钟每时比标准时间慢3分.将两个钟同时调到标准时间,结果在24时内,快钟显示9点整时,慢钟恰好显示8点整.此时的标准时间是__________.15.第四届东亚男足邀请赛共有四支足球队进行单循环赛,即每两队之间都要进行一场比赛,每场比赛胜者得3分,负者得0分,平局两队各得1分．比赛完成之后各队得分是四个连续的自然数,请计算出输给第一名的球队的得分是__________分．16.一个圆周长70厘米,甲乙两只爬虫从同一点同时出发,同向爬行,甲以4厘米/秒的速度不停的爬行,乙爬行15厘米厚,立即反向爬行,并且速度增加1倍,甲爬行40厘米处与乙第一次相遇.则乙爬虫原来的速度是__________.17. 有2个三位数相乘的积是一个五位数,积的后四位是1031,第一个数各个位的数字之和是10,第二个数的各个位数字之和是8,求两个三位数的和____________.18.对于一个正整数a ,如果2a 的末两位数字相同且不为0,则称a 是一个“少年数”.那么不超过2015的“少年数”有___________个.19. 如下图,正方形ABCD 的面积为1,E 、F 分别为BC 、CD 的中点,AE 和BF 相交于点O ．则COD △的面积是___________．OFEDCBA20. 对于数列n a a a ,...,,21,进行一次操作,变为n a a a a a a a a a ++++++ 21321211,,,,.例如1,3,4,5,进行一次操作变为1,4,8,13,如果再操作一次则变成了1,5,13,26.对于数列1,2,3,…,100,进行一次操作得到的数列末项记为1a ,连续两次得到的数列末项记为2a ,……,连续99次得到的数列末项记为99a ,求982199a a a a ---- 的值是______________.。

2014年美国“数学大联盟杯赛”(中国赛区)初赛五、六年级试卷

37. 将 1 ~ 9 九个数不重不漏地组成一个两位数、一个三位数、一个四位数。这三个数均能被 9 整除，并且 7、8、9 分别在这三个数中，三个数十位数字为三个连续的偶数，个位数字为三个连续的奇数。如果将四位数的千位移到两位数的百位，组成新的三个三位数，新的三位数也均能被 9 整除。那么题中最初的三位数是。 38. 如图为一个正方体有盖纸盒的示意图，在 1 ~ 30 的数中选出 7 个，在纸盒的每个面填一个数。将盒盖的两个数字相加后，三组相对面填的数均满足两两乘积相等。那么 x 处的数字有种可能。
姓名(签名)
A) 413 B) 1626 C) 21155 D) 161155 16. Which of the following figures has an odd number of sides? A) rhombus B) trapezoid C) pentagon D) hexagon 17. For how many integers from 55 to 66 is the ones digit greater than the tens digit? A) 4 B) 5 C) 10 D) 11 18. Lex buys 6 same-priced books and pays with a $50 bill. The change Lex receives is twice the price of a book. Each book costs A) $6.25 B) $7.14 C) $8.33 D) $12.50
14. If two consecutive whole numbers have a different number of digits, then their 一、选择题(每小题 5 分，答对加 5 分，答错不扣分，共 150 分，答案请填涂在答题卡上) 1. The band’s trombone plays 2013 notes, the trumpet plays 2014 notes, and the tuba plays 218 notes. That’s a total of ? notes. A) 6245 B) 6045 C) 4245 D) 645 2. The remainder when (999 999 999 + 666 666 + 333 + 1) is divided by 3 is A) 0 B) 1 C) 2 D) 3 3. 20 − 5 × 2 = 2 × ? A) 5 B) 15 C) 25 D) 30 C) 15 D) 100

美国数学大联盟杯赛试题

美国数学大联盟杯赛试题
一、选择题（每小题3分，共24分）
1.下列各式中错误的是( )
A. B.
C. D.
2.若，，则等于( )
A.5
B.6
C.8
D.9
3.在等式（）中，括号里填入的代数式应当是( )
A. B. C. D.
4.计算的结果为（）
A. B. C. D.
5. 下列4个算式中,计算错误的有( )
(1) (2) (3) (4)
A.4个
B.3个
C.2个
D.1个
6.如果, ,那么三数的大小为( )
A. B. C. D.
7.计算的结果为( )
A. B. C. D.
8.已知是大于1的自然数,则等于( )
A. B. C. D.
二、填空题（每空2分，共20分）
9.最薄的金箔的厚度为，用科学记数法表示为_________ ；每立方厘米的空气质量约为，用小数把它表示为.
10. ；；.
11. ；.
12.( ) ；.
13.已知：•，
若（为正整数），则.
三、解答题（共56分）
14.计算（每小题4分，共20分）：
(1) (2) (3)
15.（8分）先化简，再求值：，其中.
16.（8分）已知,求的值.
17.（10分）已知用含有的代数式表示 .
18.（10分）已知请用“>”把它们按从小到大的顺序连接起来，并说明理由.
第八章幂的运算单元自测题参考答案
三、解答题：14.（1）（2）（3）（4）（5）15.
16. 17. 18.。

六年级美国大联盟几何专题讲义(含题目翻译答案解析)

六年级美国大联盟第一阶段-几何专题（教师版）学生/课程年级学科授课教师日期时段核心内容美国大联盟几何专题课型一对N教学目标多边形的性质、周长、面积以及内角和，正方体长方体的棱长和、表面积、体积。

重、难点几何模型的应用导学一：多边形知识点讲解 1、多边形的认识专业词汇总结：rectangle：矩形 trapezoid：梯形 rhombus：菱形 pentagon：五边形hexagon：六边形 parallelogram：平行四边形 square：正方形例题1.[单选题] [平面图形的分类及识别] [难度：★ ] （2015年第7题）Which of the following is not a parallelogram?A、rectangleB、trapezoidC、rhombusD、square【参考答案】B【题目解析】翻译：下列哪个不是平行四边形？A、矩形B、梯形C、菱形D、正方形2.[单选题] [平面图形的分类及识别] [难度：★ ] （2013年第16题）Which of the following figures has an odd number of sides?A、rhombusB、trapezoidC、pentagonD、hexagon【参考答案】C【题目解析】翻译：下列哪个图形有奇数条边？A、菱形B、梯形C、五边形D、六边形3.[单选题] [平面图形的分类及识别;2、3、5的倍数特征] [难度：★★★ ] （2016年第28题）Each side of square S, pentagon P,and hexagon H is an integer.If the perimeter of each figure is the same,the perimeter could beA、2020B、2030C、2040D、2050【参考答案】C【题目解析】翻译：正方形S、五边形P、六边形H的边长都是整体，如果它们的周长相等，那么它们的周长可能是我爱展示1.[单选题] [平面图形的分类及识别] [难度：★ ] A trapezoid has sides.A、3B、4C、5D、10【参考答案】B【题目解析】翻译：梯形有多少条边？2.[单选题] [平面图形的分类及识别] [难度：★ ] Five pentagons have sides.A、20B、25C、30D、35【参考答案】A【题目解析】翻译：五个正方形有多少条边？3.[单选题] [平面图形的分类及识别] [难度：★ ] All together, 27 trapezoids have the same number of sides astriangle.A、16B、18C、27D、36【参考答案】D【题目解析】翻译：27个梯形的边数与多少个三角形的边数相同？知识点讲解长方形与正方形的面积与周长专业词汇总结：equilateral triangle：等边三角形 perimeter：周长rectangle：长方形side-length：边长length：长area：面积width：宽例题1. [单选题] [正方形的周长;三角形的周长;长方形、正方形的面积] [难度：★★★ ] （2016 年第 9 题）A square and equilateral triangle have the same side-lengths ,and the total of their perimeters is 42. What is the area of the square?A、6B、24C、36D、42【参考答案】C【题目解析】翻译：正方形和等边三角形的边长相同，它们的边长总和为42，正方形的面积是多少？2. [单选题] [正方形的周长;长方形、正方形的面积] [难度：★★★ ] 2. （2015 年第 13 题）The quotient of the square of the perimeter of square S divided by the area of square S isA、1B、2C、4D、16【参考答案】D【题目解析】翻译：正方形周长的平方除以它的面积结果是多少？3. [单选题] [长方形的周长;正方形的周长] [难度：★★★ ] （2013 年第 8 题）Four 2 × 8 rectangles havea total area equal to that of a square of perimeterA、8B、16C、32D、64【参考答案】C【题目解析】翻译：由四个2×8的矩形组成正方体，其周长为？我爱展示1.[单选题] [正方形的周长] [难度：★★★ ] Four identical squares ,each with a perimeter of 36, are put together as shown to make one big square. What is the perimeter of the big square?A、36B、48C、72D、144【参考答案】C【题目解析】翻译：如图所示，将四个相同的正方形（每个正方形的周长为36）组合在一起，形成一个大正方形。

第15届地方初赛6年级a卷

世界奥林匹克数学竞赛（中国区）选拔赛第 15 届世界奥林匹克数学竞赛（中国区）选拔赛5.如图，是一个正方体的表面展开图，折叠成正方体后与“国”字所在面相对的面的字是（）。

A.全B.学C.竞D.赛---------------------------------------------------------------------------------考场、线学校准考证号父母姓名、联系电话_装订姓名年级---------------------------------------赛区考生须知：1. 每位考生将获得考卷一份。

考试期间，不得使用计算工具或手机。

2. 本卷共 120 分，选择题为单选，每小题 4 分，共 64 分；计算题每小题 4 分，共 16 分；解答题每小题 10 分，共 40 分。

3. 请将答案写在本卷上。

考试完毕时，考卷及草稿纸会被收回。

4. 若计算结果是分数，请化至最简，并确保为真分数或带分数。

六年级地方晋级赛初赛 A 卷（本试卷满分 120 分，考试时间 90 分钟）一、选择题。

（本题为单选题，请将答案填入下图的方框中。

每题 4 分，共 64 分）题号 1 2 3 4 5 6 7 8 9 10 11 12 13 14 15 16答案1.有一种方便面的包装袋上明确标记，一袋方便面的标准重量是 120 克，合格范围是±5 克，那么下面几袋方便面重量中不合格的是（）克。

A.115B.117C.125D.1102.欧欧用方格纸做了许多英文字母的卡片，图中“D”的面积占整张卡片面积的（）。

A.3%B.10%C.30%D.70%第 2 题图第 4 题图第 5 题图第 6 题图3.将一个圆柱形纸筒的侧面沿直线剪开，不可能出现的图形是（）。

A.长方形B.三角形C.正方形D.平行四边形4.某公司对客户的满意度进行了一次抽样调查，根据统计得到的条形统计图绘制成如图所示的扇形统计图，那原来的条形统计图可能是（）。

2018年美国“数学大联盟杯赛”（中国赛区）初赛四年级试卷（1）

2018年美国“数学⼤联盟杯赛”（中国赛区）初赛四年级试卷（1）2017-2018年度美国“数学⼤联盟杯赛”(中国赛区)初赛(四年级)(初赛时间：2017年11⽉26⽇，考试时间90分钟，总分200分)学⽣诚信协议：考试期间，我确定没有就所涉及的问题或结论，与任何⼈、⽤任何⽅式交流或讨论，我确定我所填写的答案均为我个⼈独⽴完成的成果，否则愿接受本次成绩⽆效的处罚。

请在装订线内签名表⽰你同意遵守以上规定。

考前注意事项：1. 本试卷是四年级试卷，请确保和你的参赛年级⼀致；2. 本试卷共4页(正反⾯都有试题)，请检查是否有空⽩页，页数是否齐全；3. 请确保你已经拿到以下材料：本试卷(共4页，正反⾯都有试题)、答题卡、答题卡使⽤说明、英⽂词汇⼿册、草稿纸。

考试完毕，请务必将英⽂词汇⼿册带回家，上⾯有如何查询初赛成绩、及如何参加复赛的说明。

其他材料均不能带⾛，请留在原地。

选择题：每⼩题5分，答对加5分，答错不扣分，共200分，答案请填涂在答题卡上。

1.Which of the following is the smallest?A) 2.018 B) 20.18 C) 0.218 D) 20182.What is the least common multiple of 20 and 18?A) 90 B) 180 C) 240 D) 3603.The sum of the degree-measures of the exterior angles of a triangle is?A) 180 B) 360 C) 540 D) 7204.In the figure on the right, please put the numbers 1 – 11 in the elevencircles so that the three numbers in every straight line add up to 18.What is the number in the middle circle? Note: There are 5 straightlines in total in this figure.A) 6 B) 7 C) 8 D) 95.I am a lovely cat. When I multiply the digits of a whole numberand the product I get is 8, I put that whole number on my list offavorite numbers. Of the whole numbers from 1000 to 9999,how many would I put on my list of favorite numbers?A) 10 B) 12 C) 16 D) 206.Two planes take off at the same time from the same point to race to apoint and back. Place A travels at 180 miles per hour on the way out and240 miles per hour on the return trip. Plane B covers the entire distance at an averagespeed of 210 miles per hour. Which plane wins the race, or is it a tie?A) plane A wins B) plane B winsC) a tie D) non-deterministic 7.52 × 88 = 44 ×?A) 102 B) 96 C) 104 D) 1248.What is the smallest whole number that leaves a remainder of 4, 5, 6 when divided byeach of 5, 6, 7?A) 29 B) 209 C) 210 D) 20099.In △ABC, m∠A + m∠C = m∠B. What is the degree measure of ∠B?A) 80 B) 90 C) 100 D) 18010.I bought a toy for $10, sold it for $20, rebought it for $30, and resold it for $40. My totalprofit on the 4 transactions was ?A) 10 B) 20 C) 30 D) 4011.What is the greatest number of integers I can choose from the first ten positive integers sothat any 3 of the chosen integers could be the lengths of the three sides of a triangle?A) 4 B) 5 C) 6 D) 712.How many whole numbers between 200 and 400 have all their digits increasing in valuewhen read from left to right?A) 30 B) 36 C) 42 D) 4813.What is the value of 1% of 10% of 100?A) 0.01 B) 0.1 C) 1 D) 1014.If three cats can eat three bowls of food in three minutes, how many minutes will it take100 cats to eat 100 bowls of food?A) 1 B) 3C) 100 D) None of the above15.There are three squares. The area of the smallest one is 2. The side-length of the secondsquare is twice the side-length of the smallest one. And the side-length of the third square is three-times the side-length of the smallest one. The total area of the three squares isA) 12 B) 28 C) 36 D) 7216.A man, who had been married for three years, spent25of his yearly income on his family,14on business, and110on personal travel. If he saved $45000 during those three years, what was his annual income?A) $45000 B) $50000C) $65000 D) None of the above17.Given four different integers, at most how many different sums can be formed bychoosing two, three, or four of them and finding each sum?A) 8 B) 9 C) 10 D) 1118. Max places 100 eggs in 10 baskets, with each basket receiving at least1 egg, but no2 baskets receiving the same number of eggs. What is the greatest number of eggs that may be placed in a basket?A) 45 B) 47 C) 55 D) 6519. 2 + 3 × 4 – 5 =A) 0 B) 6 C) 9 D) 15 20. What is the highest power of 2 that divides 2 × 4 × 6 × 8 × 10? A) 25 B) 27 C) 28 D) 215 21. Which of the following is a prime number?A) 2017B) 2018C) 2015D) 201622. What is the greatest possible number of acute angles in a figure consisting of a triangleand a line passing through two sides of the triangle?A) 5B) 6C) 7D) 823. Amy can solve 5 questions every 3 minutes. Kate can solve 3 questions every 5 minutes.How many more questions Amy can solve than Kate in one hour?A) 15B) 32C) 60D) 6424. Using 3 Ts and 2 Js, in how many different orders can the five letters be arranged? Forexample, TTTJJ and TTJJT are two such different orders.A) 2B) 10C) 20D) 6025. Coastal Coconuts can divide all their coconuts evenly among 8, 9, or10 customers, with 1 coconut left over each time. If Coastal Coconuts has more than 1 coconut, what is the least number of coconuts they could have?A) 561 B) 721C) 831 D) None of the above 26. 35 ÷ 32 =A) 3 B) 9 C) 27 D) 81 27. If the sum of three prime numbers is 30, what is the least prime number?A) 2B) 3C) 5D) 728. Juxtaposing two identical squares to form a rectangle, the perimeter of the rectangle is 12less than the sum of the perimeter of the two squares. What is the side-length of the original square?A) 3B) 6C) 9D) 1229. It takes Mike 2 hours to finish a task. It takes 4 hours for Tom to finish the same task.Mike and Tom worked together on this task for one hour before Mike had to leave. How long will it take Tom to finish the rest of the task?A) 1 B) 2 C) 3 D) 4 30. The number of triangles in the figure on the right isA) 9 B) 10 C) 11 D) 12 31. What is the thousands digit of the product 1234560 × 2345670 × 3456780?A) 8B) 6C) 5D) 032. The sum of nine consecutive positive integers is always divisible byA) 2B) 5C) 7D) 933. You can put as many as 96 books in 6 backpacks. How many backpacks are necessary forA) 7B) 8C) 9D) 1034. The number of nickels I have is twice the number of dimes I have, and together thesecoins are worth more than $1. The least number of dimes that I can have isA) 5B) 6C) 8D) 1035. The ages of four kids are four consecutive positive integers. The product of their ages is3024. How old is the oldest kid?A) 8B) 9C) 10D) 1136. In the Game of Life, you earn 3 points for flipping a coin to “heads”, and 5 points forflipping a coin to “tails”. In all, how many positive whole number scores are IMPOSSIBLE to get after flipping it one or more times?A) 4B) 5C) 7D) 1137. Four monkeys can eat four bags of peanuts in three minutes. How many monkeys will ittake to eat 100 bags of peanuts in one hour?A) 4 B) 5 C) 20 D) 100 38. The tens digit of the product of the first 100 positive integers isA) 2B) 4C) 8D) 039. Someone put three dimes into my pile of quarters. If I add up the value of these coins,including the dimes, the sum could beA) $6.25B) $7.75C) $8.0540. Brooke's empty tub fills in 20 minutes with the drain plugged, and her full tub drains in 10 minutes with the water off. How many minutes would it take the full tub to drain while the water is on?A) 12B) 15 C) 20 D) 30。

2015年第13届“走美杯”小学数学竞赛试卷(六年级初赛B卷)

2015年第13届“走美杯”小学数学竞赛试卷（六年级初赛B卷）一、填空题（共5小题，每小题8分，满分40分）1．（8分）计算：++=．2．（8分）某商品今年的生产成本比去年增加了5%，扔保持原来的销售价格，则每件产品的利润下降了20%，那么，如果要保持成本在销售价格中所占的百分比，销售价格应该在去年的基础上提高%．3．（8分）用1，2，3，4，5，6，7，8这八个数字给4名男生与4名女生编号，要求是男生用奇数，女生用偶数，那么，一共有种不同的编号方法．4．（8分）用2015减去它的，再减去余下的，再减去余下的，…，以此类推，一直减去余下的，那么最后的得数为．5．（8分）“24点游戏”很多人熟悉的数学游戏，游戏过程如下：任意从52张扑克牌（不包括大小王）中抽取4张，用这4张扑克牌上的数字（A=1，J=11，Q=12，K=13）通过加减乘除四则运算得出24，最先找到算法者获胜．游戏规定4张牌扑克都要用到，而且每张牌只能用1次，比如2，3，4，Q．则可以由算法（2×Q）×（4﹣3）得到24．王亮在一次游戏中抽到了4，4，7，7，经过思考．他发现（4﹣）×7=24．我们将满足（a﹣）×b=24的牌组{a，a，b，b}称为“王亮牌组”，请再写出一组不同的“王亮牌组”．二、填空题（共5小题，每小题10分，满分50分）6．（10分）在荷兰的小镇卡茨林赫弗尔2013年6月建成了一个由三个半圆组成的城市雕塑，三个半圆的直径分别为24.2m，19.3m，4.9m．这个雕塑的原始图形来自于阿基米德《引理集》中的鞋匠刀形（Arbelos），即图中的阴影部分所示的图形．那么该城市雕塑中的鞋匠刀形的周长为（圆周率用π表示）．7．（10分）“足球”可以近似地看成是由一些五边形与正六边形组成的几何体，每一个顶点处有3条棱，这个几何体是阿基米德立体（Archimedean Solids）中的一个，通常，可以通过如图所示的方法，截正二十面体得到“足球”，那么，一个“足球”的棱数为．8．（10分）如图所示，BD，CE分别是∠ABC的角平分线，如果∠BAC=62°，那么，∠BFC=°．9．（10分）将图中的边染色，要求有共同顶点的两个相邻的边染不同的颜色，则至少需要中颜色．10．（10分）索马里方体是丹麦物理学家皮特•海音（Piet Hein）发明的7个小立方体组块（如图所示），如果假设这些小立方体的边长为1，则利用这7个组块不仅可以组成一个3×3的立方体，还可以组成很多美妙的几何体，那么，要组成下面的几何体，需要用到的3个索玛立方体的编号是．三、填空题（共5小题，每小题12分，满分60分）11．（12分）一个大于0的自然数如果满足所有因数（即约数）之和等于它自身的2倍，则称这个数为完全数（或完美数），比如，最小的完全数是6，因为6的所有因数为1，2，3，6，而1+2+3+6=12．古希腊时代的人们就已经认识完全数，并且找到了前4个6，28，496，8128完全数，那么，8128的全体质因数为．12．（12分）只能被1和自身整除的大于1的自然数叫做质数或素数．比如2，3，5，7，11等，如果将117分拆成10个质数之和，要求其中最大的质数尽可能大，那么，这个最大的质数为．13．（12分）我们可以将全体正整数和正分数按照如图所示的方法，从1开始，一层一层地“生长”出来；是第一层；第二层是，，第三层是，，，，…按照这个规律，在第层．14．（12分）如果两个自然数的积被13除余1，那么我们称这两个自然数互为“模13的倒数”比如，2×7=14，被13除余1，则2和7互为“模13的倒数”；1×1=1，则1的“模13的倒数”是它自身．显然，一个自然数如果存在“模13的倒数”则它的倒数并不是唯一的，比如，14就是1的另一个“模13的倒数”．判断1，2，3，4，5，6，7，8，9，10，11，12是否有“模13的倒数”，并利用所得结论计算1×2×3×4×5×6×7×8×9×10×11×12（记为12！，读作12的阶乘）被13除所得的余数．15．（12分）如果一个正方形能够被分割为若干个边长不等的小正方形，则这个正方形称为完美正方形．下面的正方形是已知包含21个小正方形的完美正方形（称为21阶完美正方形），这是迄今为止知道的最小阶数的完美正方形．分割方法如图所示，其中小正方形中心的数字代表其边长，请计算这个完美正方形的边长，并写在这里．2015年第13届“走美杯”小学数学竞赛试卷（六年级初赛B卷）参考答案与试题解析一、填空题（共5小题，每小题8分，满分40分）1．（8分）计算：++=．【分析】先把算式拆分为×+×+×，再根据乘法的分配律简算即可．【解答】解：++=×+×+×=×（++）=×1=；故答案为：．【点评】此题重点考查了学生对分数的拆项和运算定律的掌握与运用情况，要结合数据的特征，灵活选择简算方法．2．（8分）某商品今年的生产成本比去年增加了5%，扔保持原来的销售价格，则每件产品的利润下降了20%，那么，如果要保持成本在销售价格中所占的百分比，销售价格应该在去年的基础上提高5%．【分析】要使成本在销售价格中所占的百分比不变，设去年的成本为a，销售价格为b，去年成本与销售价格的百分比，即为：，设须提高去年售价的x，则可列关系式，求解即可．【解答】解：根据分析，设去年的成本为a，销售价格为b，去年成本与销售价格的百分比，即为：，销售价格在去年的基础上提高x，则有：，解得：x=5%，即：销售价格应该在去年的基础上提高5%．故答案是：5%．【点评】本题考查了利润利息和纳税的问题，本题突破点是：设成本在销售价格中所占的百分比，列出关系式，求解即可得出．3．（8分）用1，2，3，4，5，6，7，8这八个数字给4名男生与4名女生编号，要求是男生用奇数，女生用偶数，那么，一共有576种不同的编号方法．【分析】按题意，男生用奇数编号，有四个奇数，每个人有四个选择，故将四个奇数与四名男生进行排列，有4×3×2×1=24种编号方法，同理女生的编号方法利用排列的性质也可以求得，故总的编号方法不难求得．【解答】解：根据分析，男生用奇数编号，有四个奇数，每个人有四个选择，故将四个奇数与四名男生进行排列，共有：4×3×2×1=24种编号方法；女生用偶数编号，共有4个偶数编号，故四个偶数与四个女生进行一一排列，共有：4×3×2×1=24种不同的编号方法，一共有：24×24=576种不同编号方法．故答案是：576．【点评】本题考查了排列组合，突破点是：利用排列和组合，以及奇数偶数的个数，求得总的不同的编号方法．4．（8分）用2015减去它的，再减去余下的，再减去余下的，…，以此类推，一直减去余下的，那么最后的得数为65．【分析】把每次减少前的数看作单位“1”，则分别剩下单位“1”的（1﹣）、（1﹣）、（1﹣）、…、（1﹣），然后根据分数乘法的意义，用2015乘这些分率即可解决问题．【解答】解：2015×（1﹣）×（1﹣）×（1﹣）×…×（1﹣）=2015×=2015×=65故答案为：65．【点评】本题关键是确定每次剩余它前面的几分之几，计算中要根据规律约分巧算．5．（8分）“24点游戏”很多人熟悉的数学游戏，游戏过程如下：任意从52张扑克牌（不包括大小王）中抽取4张，用这4张扑克牌上的数字（A=1，J=11，Q=12，K=13）通过加减乘除四则运算得出24，最先找到算法者获胜．游戏规定4张牌扑克都要用到，而且每张牌只能用1次，比如2，3，4，Q．则可以由算法（2×Q）×（4﹣3）得到24．王亮在一次游戏中抽到了4，4，7，7，经过思考．他发现（4﹣）×7=24．我们将满足（a﹣）×b=24的牌组{a，a，b，b}称为“王亮牌组”，请再写出一组不同的“王亮牌组”（2，2，13，13），（3，3，9，9），（6，6，5，5），（8，8，4，4），（12，12，3，3）．【分析】先根据“王亮牌组”的特征，得出a，（b﹣1）是24的约数，最后借助扑克牌的特点即可得出结论．【解答】解：依题意可知：根据（a﹣）×b==a（b﹣1）=24；那么a和（b﹣1）就是24的约数；显然（a，b）的数字组合为（2，13），（3，9），（6，5），（8，4），（12，3）（扑克中最大为13）故答案为：（2，2，13，13），（3，3，9，9），（6，6，5，5），（8，8，4，4），（12，12，3，3）．【点评】此题是填符号组算式，主要考查了约数，以及理解“王亮牌组”的特点，得出a，（b﹣1）是24的约数是解本题的关键．二、填空题（共5小题，每小题10分，满分50分）6．（10分）在荷兰的小镇卡茨林赫弗尔2013年6月建成了一个由三个半圆组成的城市雕塑，三个半圆的直径分别为24.2m，19.3m，4.9m．这个雕塑的原始图形来自于阿基米德《引理集》中的鞋匠刀形（Arbelos），即图中的阴影部分所示的图形．那么该城市雕塑中的鞋匠刀形的周长为24.2π（圆周率用π表示）．【分析】显然，阴影部分的周长由三个圆的半圆弧组成的，故图中的阴影部分所示的图形那么该城市雕塑中的鞋匠刀形的周长可以用三个圆的半圆周长公式即可求得．【解答】解：根据分析，阴影部分的周长由三个圆的半圆弧组成的，故图中的阴影部分所示的图形那么该城市雕塑中的鞋匠刀形的周长===24.2π故答案是：24.2π．【点评】本题考查了圆的周长，突破点是：利用圆的周长公式不难求得阴影部分的周长．7．（10分）“足球”可以近似地看成是由一些五边形与正六边形组成的几何体，每一个顶点处有3条棱，这个几何体是阿基米德立体（Archimedean Solids）中的一个，通常，可以通过如图所示的方法，截正二十面体得到“足球”，那么，一个“足球”的棱数为90．【分析】可以根据多面体的顶点V，面数F，棱数E之间关系式V+F﹣E=2，先求出正五边形和正六边形的个数，再求棱数．【解答】解：根据分析，设多面体的顶点V，面数F，棱数E之间关系式为：V+F ﹣E=2；设有正五边形x个，正六边形y个，由题意得：解得：x=12，y=20．则棱的总数为E=（5×12+6×20）=90．故答案是：90．【点评】本题考查了组合图形的计数，突破点是：根据关系式V+F﹣E=2，先求出正五边形和正六边形的个数，再求棱数．8．（10分）如图所示，BD，CE分别是∠ABC的角平分线，如果∠BAC=62°，那么，∠BFC=121°．【分析】根据三角形的内角和，得知：∠A+∠ABC+∠ACB=180°⇒∠A+2∠FBC+2∠FCB=180°⇒∠FBC+∠FCB=（180°﹣∠A）==59°．【解答】解：根据分析，根据三角形的内角和，得知：∠A+∠ABC+∠ACB=180°⇒∠A+2∠FBC+2∠FCB=180°⇒∠FBC+∠FCB=（180°﹣∠A）==59°又∵∠BFC=180°﹣∠FBC﹣∠FCB=180°﹣59°=121°．故答案是：121°．【点评】本题考查了长度和角度，突破点是：根据三角形的内角和以及角平分线的性质，可以求得∠BFC．9．（10分）将图中的边染色，要求有共同顶点的两个相邻的边染不同的颜色，则至少需要3中颜色．【分析】首先分析内五边形的染色最低需要3色，那么只要枚举出3色可以染出来此图即可．【解答】解：依题意可知：首先分析内5点是循环的用数字代表颜色即是至少是12123的情况为3种颜色．如图所示3种颜色可以完成此图的染色．故答案为：3【点评】本题考查对染色问题的理解和运用，关键问题是找到内五边形的最低染色的标准，问题解决．10．（10分）索马里方体是丹麦物理学家皮特•海音（Piet Hein）发明的7个小立方体组块（如图所示），如果假设这些小立方体的边长为1，则利用这7个组块不仅可以组成一个3×3的立方体，还可以组成很多美妙的几何体，那么，要组成下面的几何体，需要用到的3个索玛立方体的编号是1号，3号，5号或1号，3号，6号．【分析】首先确定目标图形需要多少块单位立方体（棱长为1的立方体），由题意可知需要11块，索玛立方体的1号包含3个单位立方体，2号到7号包含4个单位立方体，而目标图形需要11个单位立方体，只能是3+4+4，所以1好必须选择，之后通过观察即可解决问题．【解答】解：首先确定目标图形需要多少块单位立方体（棱长为1的立方体），由题意可知需要11块，索玛立方体的1号包含3个单位立方体，2号到7号包含4个单位立方体，而目标图形需要11个单位立方体，只能是3+4+4，所以1好必须选择，之后通过观察可知，1号，3号，5号或1号，3号，6号是成立的．（3号放在最底层，且保持图中的姿势，1好放在3号上面）．故答案为1号，3号，5号或1号，3号，6号．【点评】本题考查剪切拼接、索马里方体，解题的关键是利用数形结合的思想思考问题，学会观察、尝试、动手操作解决问题．三、填空题（共5小题，每小题12分，满分60分）11．（12分）一个大于0的自然数如果满足所有因数（即约数）之和等于它自身的2倍，则称这个数为完全数（或完美数），比如，最小的完全数是6，因为6的所有因数为1，2，3，6，而1+2+3+6=12．古希腊时代的人们就已经认识完全数，并且找到了前4个6，28，496，8128完全数，那么，8128的全体质因数为1，2，4，8，16，32，64，127，254，508，1016，2032，4064，8128．【分析】首先是分解质因数，计算共有多少个约数．同时成组寻找即可．【解答】解：8128=26×127．因数个数（6+1）×（1+1）=14个，8128=1×8128=2×4064=4×2032=8×1016=16×508=32×254=64×127．故答案为：1，2，4，8，16，32，64，127，254，508，1016，2032，4064，8128．【点评】要想找到所以的因数关键在于计算出所有的因数个数然后按照顺序成对写出．12．（12分）只能被1和自身整除的大于1的自然数叫做质数或素数．比如2，3，5，7，11等，如果将117分拆成10个质数之和，要求其中最大的质数尽可能大，那么，这个最大的质数为97．【分析】117分成最小的9个2，第十个数也是小于100的，可以从100以内最大的质数开始枚举．【解答】解：100以内最大的质数是97，117﹣97=20，将20分拆成9的质数的和20=2×7+3×2正好符合题意．故答案为：97．【点评】首先要熟记100以内的25个质数，最大是97，尝试枚举即可，同时要注意本题可以是重复数字．13．（12分）我们可以将全体正整数和正分数按照如图所示的方法，从1开始，一层一层地“生长”出来；是第一层；第二层是，，第三层是，，，，…按照这个规律，在第9层．【分析】首先发现数阵图的规律是数阵图的规律是上边数字的乘积是下方的数字．同时发现的和是8，在相乘的分数中8即是小数3分子又是大数5的分母．枚举即可．【解答】解：依题意可知：根据数阵图规律可知；；；；数阵图的规律是上边数字的乘积是下方的数字．同时发现的和是8，在相乘的分数中8即是小数3分子又是大数5的分母．那么对应相乘的数字就是．那么他们前一个数字就是．×=．可知是第七行．是第八行，和即再第九行．故答案为：9【点评】本题考查对数阵图的理解和运用，关键是找到数字的变化规律．问题解决．14．（12分）如果两个自然数的积被13除余1，那么我们称这两个自然数互为“模13的倒数”比如，2×7=14，被13除余1，则2和7互为“模13的倒数”；1×1=1，则1的“模13的倒数”是它自身．显然，一个自然数如果存在“模13的倒数”则它的倒数并不是唯一的，比如，14就是1的另一个“模13的倒数”．判断1，2，3，4，5，6，7，8，9，10，11，12是否有“模13的倒数”，并利用所得结论计算1×2×3×4×5×6×7×8×9×10×11×12（记为12！，读作12的阶乘）被13除所得的余数12．【分析】判断1，2，3，4，5，6，7，8，9，10，11，12是否有“模13的倒数”只需从定义出发判断即可；计算1×2×3×4×5×6×7×8×9×10×11×12被13除所得的余数需要用同余的性质2来简化运算．【解答】解：观察1，2，3，4，5，6，7，8，9，10，11，12易发现：2×7=14 14÷13=1 (1)3×9=27 27÷13=2 (1)4×10=40 40÷13=3 (1)5×8=40 40÷13=3 (1)6×11=66 66÷13=5 (1)12×12=144 144÷13=11 (1)所以1，2，3，4，5，6，7，8，9，10，11，12都有“模13的倒数”．由同余的性质2可知：对于同一个除数，两个数的乘积与他们的余数的乘积同余，则：1×2×3×4×5×6×7×8×9×10×11×12=1×2×7×3×9×4×10×5×8×6×11×12=14×27×40×40×66×1214×27×40×40×66×12≡12（mod13）所以，1×2×3×4×5×6×7×8×9×10×11×12被13除所得的余数为12．答：1，2，3，4，5，6，7，8，9，10，11，12有“模13的倒数”；1×2×3×4×5×6×7×8×9×10×11×12被13除所得的余数为12．【点评】本题主要考察同余的性质2，但在运用同余性质2时，需要观察并找到2×7，3×9，…，6×11，刚好都是1×2×3×4×5×6×7×8×9×10×11×12的因式这一规律，方可解题．15．（12分）如果一个正方形能够被分割为若干个边长不等的小正方形，则这个正方形称为完美正方形．下面的正方形是已知包含21个小正方形的完美正方形（称为21阶完美正方形），这是迄今为止知道的最小阶数的完美正方形．分割方法如图所示，其中小正方形中心的数字代表其边长，请计算这个完美正方形的边长，并写在这里112．【分析】根据小正方形中心的数字代表其边长，求出变成是50、35、27的三个小正方形的边长的和，即可求出这个完美正方形的边长是多少即可．【解答】解：根据分析，根据小正方形中心的数字代表其边长，求出变成是50、35、27的三个小正方形的边长的和，即：50+35+27=85+27=112．故答案是：112．【点评】本题考查了剪切和拼接，突破点是：根据小正方形中心的数字代表其边长，求出变成是50、35、27的三个小正方形的边长的和．。

数学思维(高中)：2015-2016年度美国“数学大联盟”思维探索十至十二年级试卷(含参考答案)

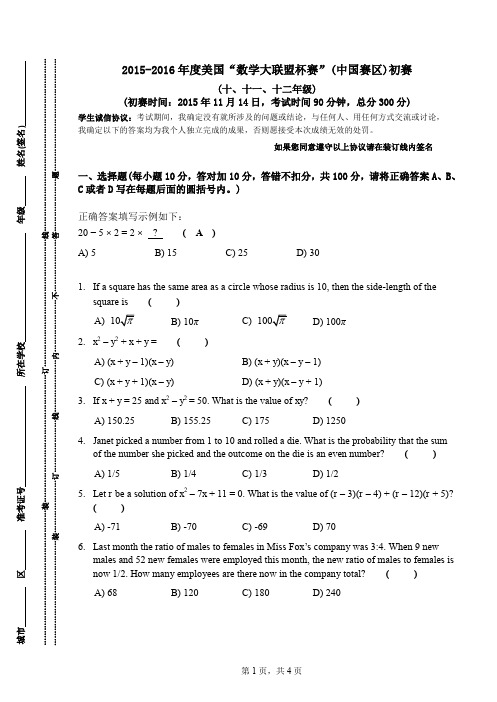
2015-2016年度美国“数学大联盟杯赛”(中国赛区)初赛(十、十一、十二年级)(初赛时间：2015年11月14日，考试时间90分钟，总分300分)学生诚信协议：考试期间，我确定没有就所涉及的问题或结论，与任何人、用任何方式交流或讨论，我确定以下的答案均为我个人独立完成的成果，否则愿接受本次成绩无效的处罚。

如果您同意遵守以上协议请在装订线内签名一、选择题(每小题10分，答对加10分，答错不扣分，共100分，请将正确答案A、B、C或者D写在每题后面的圆括号内。

)正确答案填写示例如下：20 − 5 × 2 = 2 ×? ( A )A) 5 B) 15 C) 25 D) 301.If a square has the same area as a circle whose radius is 10, then the side-length of thesquare is ( )A) B) 10πC) D) 100π2.x2–y2 + x + y = ( )A) (x + y– 1)(x–y) B) (x + y)(x–y– 1)C) (x + y + 1)(x–y) D) (x + y)(x–y + 1)3.If x + y = 25 and x2–y2 = 50. What is the value of xy? ( )A) 150.25 B) 155.25 C) 175 D) 12504.Janet picked a number from 1 to 10 and rolled a die. What is the probability that the sumof the number she picked and the outcome on the die is an even number? ( )A) 1/5 B) 1/4 C) 1/3 D) 1/25.Let r be a solution of x2– 7x + 11 = 0. What is the value of (r– 3)(r– 4) + (r– 12)(r + 5)?( )A) -71 B) -70 C) -69 D) 70st month the ratio of males to females in Miss Fox’s company was 3:4. When 9 newmales and 52 new females were employed this month, the new ratio of males to females is now 1/2. How many employees are there now in the company total? ( )A) 68 B) 120 C) 180D) 240第1页，共4页his task, he returned 40 mph from the castle to home. What is his average speed, in mph, of his quest? ( )A) 120/7 B) 240/7 C) 35 D) 70to shoot 3 apples, then when I use up the darts, I will be left with 35apples; if each dart is used to shoot 4 apples, then when I use up the apples,I will be left with 5 darts. I have ? apples at the beginning. ( )A) 51 B) 55 C) 200 D) 2409.x/2 = y/3 = z/4, what is the value of x:y:z? ( )A) 6:4:3 B) 3:4:6 C) 2:3:4 D) 4:3:210.Super Jack and Almighty Jill were doing the 100-mile walk at the same time and samestarting point, at constant speeds. Jack took a 5-minute break at the end of every 10 miles;Jill took a 10-minute break at the end of each 20 miles. Jill’s speed was 5/8 of that of Jack.They finished at the same time. How long, in minutes, does the trip take? ( )A) 53.333 B) 56.667 C) 60.333 D) 60.667二、填空题(每小题10分，答对加10分，答错不扣分，共200分。

2014-2015年度美国”数学大联盟杯赛“(中国赛区)初赛-(十、十一、十二年级).doc

2014-2015年度美国”数学大联盟杯赛“（中国赛区）初赛（十、十一、十二年级）一、选择题（每小题10分，答对加10分，答错不扣分，共100分，请将正确答案A 、B 、C 或者D 写在每题后面的圆括号内。

）正确答案填写示例如下：=-⨯⨯20522 ？（A ）A)5 B)15 C)25 D)301. Meg loves her megaphone! The large circular end has a circumference that is the reciprocal of its diameter. What is the area of the circle? ( )A)π14 B) π12 C) 14 D) 122. How many solutions does the equation x x +=233 have? ( )A)0 B)1 C)2 D)43. If y x =-1, which of the following is always true for any value of x ? ( )A) ()()x y -=-2211B) ()()x x y y -=-222211 C) ()()x x y y --=-222211 D) ()()()()x x y y -+=-+22221111 4. Lee the crow ate a grams of feed that was 1% seed, b grams of feed that was 2% seed, and c grams of feed that was 3% seed. If combined, all the feed he ate was 1.5% seed. What is a in terms of b and c ?( )A)b c +3B)b c +3 C)b c +23 D)b c +32 5. If <x 0 and <.x 2001, then x -1 must be ( )A)less than -10B)between-0.1 and 0 C)between 0and 0.1 D) greater than 106. At 9:00 A.M., the ratio of red to black cars in a parking lot was 1 to 5. An hour later the number of red cars had increased by 2, the number of black cars had decreased by 5, and the ratio of red to black cars was 1 to 4. How many black cars were in the lot at 10:00 A.M.? ( )A)13 B)15 C)60 D)657. If x ≠1and x ≠-1, then ()()()x x x x x --++-32241111=( ) A)x -21 B) x +21 C) x -241 D) x -341 8. The Camps are driving at a constant rate. At noon they had driven 300 km.At 3:30 P.M. they had driven 50% further than they had driven by 1:30 P.M.What is their constant rate in km/hr? ( )A)150 B)120 C)100 D)909. The letters in DIGITS can be arranged in how many orders without adjacent I ’s? ( )A)240 B)355 C)600 D)71510. Al, Bea, and Cal each paint at constant rates, and together they are painting a house. Al and Bea togethercould do the job in 12 hours; Al and Cal could to it in 15, and Bea and Cal could do it in 20. How many hours will it take all three working together to paint the house?( )A)8.5 B)9 C)10 D)10.5二、填空题（每小题10分，答对加10分，答错不扣分，共200分）11. What is the sum of the degree-measures of the angles at the outer points ,,,A B C D and E of a five-pointed star, as shown? Answer: . 12. What is the ordered pair of positive integers (,k b ), with the least value of k , which satisfiesk b ⋅⋅=34234?Answer: .13. A face-down stack of 8 playing cards consisted of 4 Aces (A ’s) and 4 Kings (K ’s).After I revealed and then removed the top card, I moved the new top card to thebottom of the stack without revealing the card. I repeated this procedure until thestack without revealing the card. I repeated this procedure until the stack was leftwith only 1 card, which I then revealed. The cards revealed were AKAKAKAK ,in that order. If my original stack of 8 cards had simply been revealed one card at atime, from top to bottom (without ever moving cards to the bottom of the stack),in what order would they have been revealed?Answer: .14. For what value of a is one root of ()x a x a -+++=222120 twice the other root?Answer: .15. Each time I withdrew $32 from my magical bank account, the account ’sremaining balance doubled. No other account activity was permitted. My fifth$32 withdrawal caused my account ’s balance to become $0. With how manydollars did I open that account?Answer: .16. In how many ways can I select six of the first 20 positive integers, disregarding the order in which these sixintegers are selected, so that no two of the selected integers are consecutive integers?Answer: .17. If, for all real ,()()xx f x f x =-21, what is the numerical value of f (3)?Answer: .18. How many pairs of positive integers (without regard to order) have a least common multiple of 540?Answer: .19. If the square of the smaller of consecutive positive integers is x , what is the square of the larger of thesetwo integers, in terms of x ?Answer: .20. A pair of salt and pepper shakers comes in two types: identical and fraternal.Identical pairs are always the same color. Fraternal pairs are the same colorhalf the time. The probability that a pair of shakers is fraternal is p andthat a pair is identical is .q p =-1 If a pair of shakers is of the same color, AE DCBword 格式-可编辑-感谢下载支持 determine, in terms of the variable q alone, the probability that the pair is identical. Answer: .21. As shown, one angle of a triangle is divided into four smaller congruentangles. If the lengths of the sides of this triangle are 84, 98, and 112, as shown,how long is the segment marked x ?Answer: .22. How long is the longer diagonal of a rhombus whose perimeter is 60, if threeof its vertices lie on a circle whose diameter is 25, as shown?Answer: .23. The 14 cabins of the Titanic Mail Boat are numbered consecutively from1 through 14, as are the 14 room keys. In how many different ways canthe 14 room keys be placed in the 14 rooms, 1 per room, so that, for everyroom, the sum of that room ’s number and the number of the key placed inthat room is a multiple of 3?Answer: .24. For some constant b , if the minimum value of ()x x b f x x x b -+=++2222is 12, what is the maximum value of ()f x ? Answer: .25. If the lengths of two sides of a triangle are 60cos A and 25sin A , what is the greatest possible integer-length of the third side?Answer: .26. {}n a is a geometric sequence in which each term is a positive number. If a a =5627, what is the value oflog log log ?a a a +++3132310Answer: . 27. What is the greatest possible value of ()=sin cos ?f x x x ++3412Answer: .28. Let C be a cube. Triangle T is formed by connecting the midpoints of three edges of cube C . What is the greatest possible measure of an angle of triangle T ?Answer: .29. Let a and b be two real numbers. ()sin f x a x b x =++34 and (lg log )f =3105. What is the value of (lg lg )f 3?Answer: .30. Mike likes to gamble. He always bets all his chips whenever the number of chips he has is <=5. He always bets n （10-）chips whenever the number of chips he has is greater than 5 and less than 10. He continues betting until either he has no chips or he has more than 9 chips. For every round, if he bets n chips. The probability that he wins or loses in each round is 50%. If Mike begins with 4 chips, what is the probability that he loses all his chips?Answer: .1129884xword格式-可编辑-感谢下载支持。

(参考资料)2014年美国“数学大联盟杯赛”(中国赛区)初赛五、六年级试卷

A) 100
B) 160
C) 200
D) 250
二、填空题(每小题 5 分，答对加 5 分，答错不扣分，共 50 分，答案请填涂在答题卡上)
31. The sum of the digits of 2014 is 2 + 0 + 1 + 4 = 7. Let n be a natural number.
m = n + 2014. The sum of the digits of m is half the sum of the digits of n.
What is the minimum value of n?
Answer: ______.
32. The sum of 5 different prime numbers is 200. Each of the 5 prime
1
2
26. If 5 of the 200 stripes on Frank’s giant shell are blue, 5 of the remaining
stripes are brown, and the rest are white, there are ? more white stripes
D) 110
22. The average of 2014 sixes is equal to the average of 4028 ? .
A) threes
B) sixes
C) nines
D) twelves
23. What is 0.625% of 8% of 500?
A) 0.25
B) 2.5
数字为三个连续的偶数，个位数字为三个连续的奇数。如果将四位数的

2014年美国“数学大联盟杯赛”(中国赛区)初赛五、六年级详解

2013-2014 年度美国“数学大联盟杯赛”(中国赛区)初赛答案
(五、六级) 一、选择题 1. C. The band’s trombone plays 2013 notes, the trumpet plays 2014 notes, and the tuba plays 218 notes. That is a total of 2013 + 2014 + 218 = 4245 notes. A) 6245 B) 6045 C) 4245 D) 645 2. B. This has the same remainder as 1 divided by 3. The remainder is 1. A) 0 B) 1 C) 2 D) 3 3. A. 20 − 5 C) 25 D) 30 4. D. (2 × 2) × (2 × 3) × (2 × 4) × (2 × 5) = 2 × 3 × 4 × 5 × (2 × 2 × 2 × 2). A) 2 B) 6 C) 8 D) 16 5. A. When I split the cost of a video game equally with 4 friends, we each pay $12. It costs the five of us 5 × $12 = $60. If only 4 of us split the cost, we each pay $60 ÷ 4 = $15. We each pay $15 − $12 = $3 more. A) $3.00 B) $4.00 C) $15.00 D) $16.00 6. C. At 5:00 P.M. on Friday, Hal got locked in. Since 5040 mins. is 5040 ÷60 = 84 hrs., Hal got out in 3 days 12 hrs. That’s Tuesday at 5 A.M. A) Sunday B) Monday C) Tuesday D) Wednesday

2015年第13届“走美杯”小学数学竞赛试卷(六年级初赛B卷)

2015年第13届“走美杯”小学数学竞赛试卷（六年级初赛B卷）一、填空题（共5小题，每小题8分，满分40分）1．（8分）计算：++=．2．（8分）某商品今年的生产成本比去年增加了5%，扔保持原来的销售价格，则每件产品的利润下降了20%，那么，如果要保持成本在销售价格中所占的百分比，销售价格应该在去年的基础上提高%．3．（8分）用1，2，3，4，5，6，7，8这八个数字给4名男生与4名女生编号，要求是男生用奇数，女生用偶数，那么，一共有种不同的编号方法．4．（8分）用2015减去它的，再减去余下的，再减去余下的，…，以此类推，一直减去余下的，那么最后的得数为．5．（8分）“24点游戏”很多人熟悉的数学游戏，游戏过程如下：任意从52张扑克牌（不包括大小王）中抽取4张，用这4张扑克牌上的数字（A=1，J=11，Q=12，K=13）通过加减乘除四则运算得出24，最先找到算法者获胜．游戏规定4张牌扑克都要用到，而且每张牌只能用1次，比如2，3，4，Q．则可以由算法（2×Q）×（4﹣3）得到24．王亮在一次游戏中抽到了4，4，7，7，经过思考．他发现（4﹣）×7=24．我们将满足（a﹣）×b=24的牌组{a，a，b，b}称为“王亮牌组”，请再写出一组不同的“王亮牌组”．二、填空题（共5小题，每小题10分，满分50分）6．（10分）在荷兰的小镇卡茨林赫弗尔2013年6月建成了一个由三个半圆组成的城市雕塑，三个半圆的直径分别为24.2m，19.3m，4.9m．这个雕塑的原始图形来自于阿基米德《引理集》中的鞋匠刀形（Arbelos），即图中的阴影部分所示的图形．那么该城市雕塑中的鞋匠刀形的周长为（圆周率用π表示）．7．（10分）“足球”可以近似地看成是由一些五边形与正六边形组成的几何体，每一个顶点处有3条棱，这个几何体是阿基米德立体（Archimedean Solids）中的一个，通常，可以通过如图所示的方法，截正二十面体得到“足球”，那么，一个“足球”的棱数为．8．（10分）如图所示，BD，CE分别是∠ABC的角平分线，如果∠BAC=62°，那么，∠BFC=°．9．（10分）将图中的边染色，要求有共同顶点的两个相邻的边染不同的颜色，则至少需要中颜色．10．（10分）索马里方体是丹麦物理学家皮特•海音（Piet Hein）发明的7个小立方体组块（如图所示），如果假设这些小立方体的边长为1，则利用这7个组块不仅可以组成一个3×3的立方体，还可以组成很多美妙的几何体，那么，要组成下面的几何体，需要用到的3个索玛立方体的编号是．三、填空题（共5小题，每小题12分，满分60分）11．（12分）一个大于0的自然数如果满足所有因数（即约数）之和等于它自身的2倍，则称这个数为完全数（或完美数），比如，最小的完全数是6，因为6的所有因数为1，2，3，6，而1+2+3+6=12．古希腊时代的人们就已经认识完全数，并且找到了前4个6，28，496，8128完全数，那么，8128的全体质因数为．12．（12分）只能被1和自身整除的大于1的自然数叫做质数或素数．比如2，3，5，7，11等，如果将117分拆成10个质数之和，要求其中最大的质数尽可能大，那么，这个最大的质数为．13．（12分）我们可以将全体正整数和正分数按照如图所示的方法，从1开始，一层一层地“生长”出来；是第一层；第二层是，，第三层是，，，，…按照这个规律，在第层．14．（12分）如果两个自然数的积被13除余1，那么我们称这两个自然数互为“模13的倒数”比如，2×7=14，被13除余1，则2和7互为“模13的倒数”；1×1=1，则1的“模13的倒数”是它自身．显然，一个自然数如果存在“模13的倒数”则它的倒数并不是唯一的，比如，14就是1的另一个“模13的倒数”．判断1，2，3，4，5，6，7，8，9，10，11，12是否有“模13的倒数”，并利用所得结论计算1×2×3×4×5×6×7×8×9×10×11×12（记为12！，读作12的阶乘）被13除所得的余数．15．（12分）如果一个正方形能够被分割为若干个边长不等的小正方形，则这个正方形称为完美正方形．下面的正方形是已知包含21个小正方形的完美正方形（称为21阶完美正方形），这是迄今为止知道的最小阶数的完美正方形．分割方法如图所示，其中小正方形中心的数字代表其边长，请计算这个完美正方形的边长，并写在这里．2015年第13届“走美杯”小学数学竞赛试卷（六年级初赛B卷）参考答案与试题解析一、填空题（共5小题，每小题8分，满分40分）1．（8分）计算：++=．【分析】先把算式拆分为×+×+×，再根据乘法的分配律简算即可．【解答】解：++=×+×+×=×（++）=×1=；故答案为：．【点评】此题重点考查了学生对分数的拆项和运算定律的掌握与运用情况，要结合数据的特征，灵活选择简算方法．2．（8分）某商品今年的生产成本比去年增加了5%，扔保持原来的销售价格，则每件产品的利润下降了20%，那么，如果要保持成本在销售价格中所占的百分比，销售价格应该在去年的基础上提高5%．【分析】要使成本在销售价格中所占的百分比不变，设去年的成本为a，销售价格为b，去年成本与销售价格的百分比，即为：，设须提高去年售价的x，则可列关系式，求解即可．【解答】解：根据分析，设去年的成本为a，销售价格为b，去年成本与销售价格的百分比，即为：，销售价格在去年的基础上提高x，则有：，解得：x=5%，即：销售价格应该在去年的基础上提高5%．故答案是：5%．【点评】本题考查了利润利息和纳税的问题，本题突破点是：设成本在销售价格中所占的百分比，列出关系式，求解即可得出．3．（8分）用1，2，3，4，5，6，7，8这八个数字给4名男生与4名女生编号，要求是男生用奇数，女生用偶数，那么，一共有576种不同的编号方法．【分析】按题意，男生用奇数编号，有四个奇数，每个人有四个选择，故将四个奇数与四名男生进行排列，有4×3×2×1=24种编号方法，同理女生的编号方法利用排列的性质也可以求得，故总的编号方法不难求得．【解答】解：根据分析，男生用奇数编号，有四个奇数，每个人有四个选择，故将四个奇数与四名男生进行排列，共有：4×3×2×1=24种编号方法；女生用偶数编号，共有4个偶数编号，故四个偶数与四个女生进行一一排列，共有：4×3×2×1=24种不同的编号方法，一共有：24×24=576种不同编号方法．故答案是：576．【点评】本题考查了排列组合，突破点是：利用排列和组合，以及奇数偶数的个数，求得总的不同的编号方法．4．（8分）用2015减去它的，再减去余下的，再减去余下的，…，以此类推，一直减去余下的，那么最后的得数为65．【分析】把每次减少前的数看作单位“1”，则分别剩下单位“1”的（1﹣）、（1﹣）、（1﹣）、…、（1﹣），然后根据分数乘法的意义，用2015乘这些分率即可解决问题．【解答】解：2015×（1﹣）×（1﹣）×（1﹣）×…×（1﹣）=2015×=2015×=65故答案为：65．【点评】本题关键是确定每次剩余它前面的几分之几，计算中要根据规律约分巧算．5．（8分）“24点游戏”很多人熟悉的数学游戏，游戏过程如下：任意从52张扑克牌（不包括大小王）中抽取4张，用这4张扑克牌上的数字（A=1，J=11，Q=12，K=13）通过加减乘除四则运算得出24，最先找到算法者获胜．游戏规定4张牌扑克都要用到，而且每张牌只能用1次，比如2，3，4，Q．则可以由算法（2×Q）×（4﹣3）得到24．王亮在一次游戏中抽到了4，4，7，7，经过思考．他发现（4﹣）×7=24．我们将满足（a﹣）×b=24的牌组{a，a，b，b}称为“王亮牌组”，请再写出一组不同的“王亮牌组”（2，2，13，13），（3，3，9，9），（6，6，5，5），（8，8，4，4），（12，12，3，3）．【分析】先根据“王亮牌组”的特征，得出a，（b﹣1）是24的约数，最后借助扑克牌的特点即可得出结论．【解答】解：依题意可知：根据（a﹣）×b==a（b﹣1）=24；那么a和（b﹣1）就是24的约数；显然（a，b）的数字组合为（2，13），（3，9），（6，5），（8，4），（12，3）（扑克中最大为13）故答案为：（2，2，13，13），（3，3，9，9），（6，6，5，5），（8，8，4，4），（12，12，3，3）．【点评】此题是填符号组算式，主要考查了约数，以及理解“王亮牌组”的特点，得出a，（b﹣1）是24的约数是解本题的关键．二、填空题（共5小题，每小题10分，满分50分）6．（10分）在荷兰的小镇卡茨林赫弗尔2013年6月建成了一个由三个半圆组成的城市雕塑，三个半圆的直径分别为24.2m，19.3m，4.9m．这个雕塑的原始图形来自于阿基米德《引理集》中的鞋匠刀形（Arbelos），即图中的阴影部分所示的图形．那么该城市雕塑中的鞋匠刀形的周长为24.2π（圆周率用π表示）．【分析】显然，阴影部分的周长由三个圆的半圆弧组成的，故图中的阴影部分所示的图形那么该城市雕塑中的鞋匠刀形的周长可以用三个圆的半圆周长公式即可求得．【解答】解：根据分析，阴影部分的周长由三个圆的半圆弧组成的，故图中的阴影部分所示的图形那么该城市雕塑中的鞋匠刀形的周长===24.2π故答案是：24.2π．【点评】本题考查了圆的周长，突破点是：利用圆的周长公式不难求得阴影部分的周长．7．（10分）“足球”可以近似地看成是由一些五边形与正六边形组成的几何体，每一个顶点处有3条棱，这个几何体是阿基米德立体（Archimedean Solids）中的一个，通常，可以通过如图所示的方法，截正二十面体得到“足球”，那么，一个“足球”的棱数为90．【分析】可以根据多面体的顶点V，面数F，棱数E之间关系式V+F﹣E=2，先求出正五边形和正六边形的个数，再求棱数．【解答】解：根据分析，设多面体的顶点V，面数F，棱数E之间关系式为：V+F ﹣E=2；设有正五边形x个，正六边形y个，由题意得：解得：x=12，y=20．则棱的总数为E=（5×12+6×20）=90．故答案是：90．【点评】本题考查了组合图形的计数，突破点是：根据关系式V+F﹣E=2，先求出正五边形和正六边形的个数，再求棱数．8．（10分）如图所示，BD，CE分别是∠ABC的角平分线，如果∠BAC=62°，那么，∠BFC=121°．【分析】根据三角形的内角和，得知：∠A+∠ABC+∠ACB=180°⇒∠A+2∠FBC+2∠FCB=180°⇒∠FBC+∠FCB=（180°﹣∠A）==59°．【解答】解：根据分析，根据三角形的内角和，得知：∠A+∠ABC+∠ACB=180°⇒∠A+2∠FBC+2∠FCB=180°⇒∠FBC+∠FCB=（180°﹣∠A）==59°又∵∠BFC=180°﹣∠FBC﹣∠FCB=180°﹣59°=121°．故答案是：121°．【点评】本题考查了长度和角度，突破点是：根据三角形的内角和以及角平分线的性质，可以求得∠BFC．9．（10分）将图中的边染色，要求有共同顶点的两个相邻的边染不同的颜色，则至少需要3中颜色．【分析】首先分析内五边形的染色最低需要3色，那么只要枚举出3色可以染出来此图即可．【解答】解：依题意可知：首先分析内5点是循环的用数字代表颜色即是至少是12123的情况为3种颜色．如图所示3种颜色可以完成此图的染色．故答案为：3【点评】本题考查对染色问题的理解和运用，关键问题是找到内五边形的最低染色的标准，问题解决．10．（10分）索马里方体是丹麦物理学家皮特•海音（Piet Hein）发明的7个小立方体组块（如图所示），如果假设这些小立方体的边长为1，则利用这7个组块不仅可以组成一个3×3的立方体，还可以组成很多美妙的几何体，那么，要组成下面的几何体，需要用到的3个索玛立方体的编号是1号，3号，5号或1号，3号，6号．【分析】首先确定目标图形需要多少块单位立方体（棱长为1的立方体），由题意可知需要11块，索玛立方体的1号包含3个单位立方体，2号到7号包含4个单位立方体，而目标图形需要11个单位立方体，只能是3+4+4，所以1好必须选择，之后通过观察即可解决问题．【解答】解：首先确定目标图形需要多少块单位立方体（棱长为1的立方体），由题意可知需要11块，索玛立方体的1号包含3个单位立方体，2号到7号包含4个单位立方体，而目标图形需要11个单位立方体，只能是3+4+4，所以1好必须选择，之后通过观察可知，1号，3号，5号或1号，3号，6号是成立的．（3号放在最底层，且保持图中的姿势，1好放在3号上面）．故答案为1号，3号，5号或1号，3号，6号．【点评】本题考查剪切拼接、索马里方体，解题的关键是利用数形结合的思想思考问题，学会观察、尝试、动手操作解决问题．三、填空题（共5小题，每小题12分，满分60分）11．（12分）一个大于0的自然数如果满足所有因数（即约数）之和等于它自身的2倍，则称这个数为完全数（或完美数），比如，最小的完全数是6，因为6的所有因数为1，2，3，6，而1+2+3+6=12．古希腊时代的人们就已经认识完全数，并且找到了前4个6，28，496，8128完全数，那么，8128的全体质因数为1，2，4，8，16，32，64，127，254，508，1016，2032，4064，8128．【分析】首先是分解质因数，计算共有多少个约数．同时成组寻找即可．【解答】解：8128=26×127．因数个数（6+1）×（1+1）=14个，8128=1×8128=2×4064=4×2032=8×1016=16×508=32×254=64×127．故答案为：1，2，4，8，16，32，64，127，254，508，1016，2032，4064，8128．【点评】要想找到所以的因数关键在于计算出所有的因数个数然后按照顺序成对写出．12．（12分）只能被1和自身整除的大于1的自然数叫做质数或素数．比如2，3，5，7，11等，如果将117分拆成10个质数之和，要求其中最大的质数尽可能大，那么，这个最大的质数为97．【分析】117分成最小的9个2，第十个数也是小于100的，可以从100以内最大的质数开始枚举．【解答】解：100以内最大的质数是97，117﹣97=20，将20分拆成9的质数的和20=2×7+3×2正好符合题意．故答案为：97．【点评】首先要熟记100以内的25个质数，最大是97，尝试枚举即可，同时要注意本题可以是重复数字．13．（12分）我们可以将全体正整数和正分数按照如图所示的方法，从1开始，一层一层地“生长”出来；是第一层；第二层是，，第三层是，，，，…按照这个规律，在第9层．【分析】首先发现数阵图的规律是数阵图的规律是上边数字的乘积是下方的数字．同时发现的和是8，在相乘的分数中8即是小数3分子又是大数5的分母．枚举即可．【解答】解：依题意可知：根据数阵图规律可知；；；；数阵图的规律是上边数字的乘积是下方的数字．同时发现的和是8，在相乘的分数中8即是小数3分子又是大数5的分母．那么对应相乘的数字就是．那么他们前一个数字就是．×=．可知是第七行．是第八行，和即再第九行．故答案为：9【点评】本题考查对数阵图的理解和运用，关键是找到数字的变化规律．问题解决．14．（12分）如果两个自然数的积被13除余1，那么我们称这两个自然数互为“模13的倒数”比如，2×7=14，被13除余1，则2和7互为“模13的倒数”；1×1=1，则1的“模13的倒数”是它自身．显然，一个自然数如果存在“模13的倒数”则它的倒数并不是唯一的，比如，14就是1的另一个“模13的倒数”．判断1，2，3，4，5，6，7，8，9，10，11，12是否有“模13的倒数”，并利用所得结论计算1×2×3×4×5×6×7×8×9×10×11×12（记为12！，读作12的阶乘）被13除所得的余数12．【分析】判断1，2，3，4，5，6，7，8，9，10，11，12是否有“模13的倒数”只需从定义出发判断即可；计算1×2×3×4×5×6×7×8×9×10×11×12被13除所得的余数需要用同余的性质2来简化运算．【解答】解：观察1，2，3，4，5，6，7，8，9，10，11，12易发现：2×7=14 14÷13=1 (1)3×9=27 27÷13=2 (1)4×10=40 40÷13=3 (1)5×8=40 40÷13=3 (1)6×11=66 66÷13=5 (1)12×12=144 144÷13=11 (1)所以1，2，3，4，5，6，7，8，9，10，11，12都有“模13的倒数”．由同余的性质2可知：对于同一个除数，两个数的乘积与他们的余数的乘积同余，则：1×2×3×4×5×6×7×8×9×10×11×12=1×2×7×3×9×4×10×5×8×6×11×12=14×27×40×40×66×1214×27×40×40×66×12≡12（mod13）所以，1×2×3×4×5×6×7×8×9×10×11×12被13除所得的余数为12．答：1，2，3，4，5，6，7，8，9，10，11，12有“模13的倒数”；1×2×3×4×5×6×7×8×9×10×11×12被13除所得的余数为12．【点评】本题主要考察同余的性质2，但在运用同余性质2时，需要观察并找到2×7，3×9，…，6×11，刚好都是1×2×3×4×5×6×7×8×9×10×11×12的因式这一规律，方可解题．15．（12分）如果一个正方形能够被分割为若干个边长不等的小正方形，则这个正方形称为完美正方形．下面的正方形是已知包含21个小正方形的完美正方形（称为21阶完美正方形），这是迄今为止知道的最小阶数的完美正方形．分割方法如图所示，其中小正方形中心的数字代表其边长，请计算这个完美正方形的边长，并写在这里112．【分析】根据小正方形中心的数字代表其边长，求出变成是50、35、27的三个小正方形的边长的和，即可求出这个完美正方形的边长是多少即可．【解答】解：根据分析，根据小正方形中心的数字代表其边长，求出变成是50、35、27的三个小正方形的边长的和，即：50+35+27=85+27=112．故答案是：112．【点评】本题考查了剪切和拼接，突破点是：根据小正方形中心的数字代表其边长，求出变成是50、35、27的三个小正方形的边长的和．。

2018年美国“数学大联盟杯赛”(中国赛区)初赛六年级试卷及答案

2017-2018年度美国“数学大联盟杯赛”(中国赛区)初赛(六年级)(初赛时间：2017年11月26日，考试时间90分钟，总分200分)学生诚信协议：考试期间，我确定没有就所涉及的问题或结论，与任何人、用任何方式交流或讨论，我确定我所填写的答案均为我个人独立完成的成果，否则愿接受本次成绩无效的处罚。

请在装订线内签名表示你同意遵守以上规定。

考前注意事项：1. 本试卷是六年级试卷，请确保和你的参赛年级一致；2. 本试卷共4页(正反面都有试题)，请检查是否有空白页，页数是否齐全；3. 请确保你已经拿到以下材料：本试卷(共4页，正反面都有试题)、答题卡、答题卡使用说明、英文词汇手册、草稿纸。

考试完毕，请务必将英文词汇手册带回家，上面有如何查询初赛成绩、及如何参加复赛的说明。

其他材料均不能带走，请留在原地。

选择题：每小题5分，答对加5分，答错不扣分，共200分，答案请填涂在答题卡上。

1. Pick any integer greater than 1. Double it twice, then triple the result. The final outcomeis ? of your starting integer.A) 700%B) 1100%C) 1200%D) 1300%2. Barry listened to the radio for 3 hours and 36 minutes. Rounded to thenearest 10 minutes, for how many minutes was Barry listening? A) 210 B) 220 C) 330 D) 340 3. Divide 99 by 22 to get a quotient and remainder. Divide that remainder by that quotient, and the new remainder is A) 4 B) 3 C) 2 D) 14. A man had five pieces of chain, each made up of three links, figure below. He wanted to join the five pieces together to make a big chain of fifteen links and went to a blacksmith to see how much it would cost. “Well,” said the blacksmith, “I will charge you 50 cents for cutting a link and $1.00 for welding a link. Any bending that is required is free.” Given those prices, what is the smallest amount of money for which the job could be done? Note: In a chain, each link is connected to one or two other links. A) $4.50 B) $5.00 C) $5.50 D) $6.00 5. A bee sat on the head of a horse rider whose horse was trotting eastbound at a steady five miles per hour. Some distance ahead on the same path, another horse and rider were approaching westbound, also at five miles per hour. When the two horses were 20 miles apart, the bee left the first horse rider and flew toward the second horse at a rate of ten miles per hour. Upon reaching the second horse, the bee immediately turned around and flew back at the same rate to the first horse. If the bee kept up this performance until the two riders met, how far (in miles) did he travel from the moment he left the first horse rider?A) 10B) 20C) 30D) None of the above6. Which of the following is the sum of the prime factors of 2018?A) 11 B) 219 C) 1011 D) 20197. If the length of the longest side of a triangle is 18, which of the following could not be the length of its second-longest side? A) 9 B) 10 C) 12 D) 17 8. My final score in a competition is the average of my scores on 5 rounds. To get a final score of 88 after getting 84, 80, and 92 on the first 3 rounds, what must be my average score for the last 2 rounds? A) 88 B) 90 C) 92 D) 96 9. Mr. Rice had breakfast one day at a restaurant with Mr. Wheat. When it came time to pay the bill, it was found that Mr. Rice had as many one-dollar bills as Mr. Wheat had quarters. (Mr. Rice had one-dollar bills only, and Mr. Wheat had quarters only.) Rather than each man paying separately, Mr. Rice paid his share of the bill, $6, to Mr. Wheat. At that point, Mr. Wheat had four times as much money as Mr. Rice. How much money did Mr. Rice have at the beginning? A) $6 B) $8 C) $9 D) $12 10. Professor Peach teaches chemistry to clever kids. The ratio of freshmen to other students in his class is 3:8. The total number of students in Professor Peach’s class could be A) 42 B) 45 C) 56 D) 77 11. 440 ÷ 220 = A) 22B) 24C) 220D) 26012. Mr. Bogsworth once left a will which read:To Bob, twice as much as to Betty. To Brian, twice as much as to Bob. To Bill, twice as much as to Brian.If his estate was valued at $45000, how much money did Betty, one of his four heirs, receive? A) $1000 B) $2000 C) $3000 D) $6000 13. I paid $5 and got 5 quarters, 5 dimes, and 5 nickels in change. I spent A) $3.00 B) $3.25 C) $3.45 D) $3.75 14. One side of Todd ’s truck is a perfect rectangle with an area of 12 m 2. If its length is 3 times its width, then its perimeter is A) 8 m B) 12 m C) 16 m D) 20 m15. If a bird in the hand is worth two in the bush, and a bird in the bush is worth four in the sky, then 4 birds in the hand are worth ? birds in the sky.A) 1B) 4C) 16D) 3216. On each of the four shelves of my bookcase is a different prime number of books. Therecould be a total of ? books on my shelves.A) 15B) 21C) 22D) 24第1页，共4页第2页，共4页Seven years ago I realized that my age would be tripled twelve years from then. How old am I now? A) 11 B) 13 C) 16 D) 18How many fractions with a numerator of 1 and a whole-number denominator are greater than 0.01 and less than 1? A) 98 B) 99 C) 100 D) 101If I write the letters R-E-P-E-A-T repeatedly, stopping when I have written exactly 100 letters, how many times do I write the letter E? A) 16 B) 18 C) 32 D) 34On my map, 1 cm represents 100 km. If a park shown on the map is a rectangle that is2.5 cm by 4 cm, the area of the actual park is ? km 2. A) 100 B) 1000 C) 10 000 D) 100 000Gloomy Gus’s Tu esday rain cloud shows up every Tuesday at 8:30 A.M.and every 50 minutes after that. Its last appearance on Tuesday is at ? P.M.A) 11:00 B) 11:10 C) 11:30 D) 11:50If my lucky number divided by its reciprocal is 100, then the square ofmy lucky number isA) 100 B) 10 C) 1D) 1100A boy and his sister were walking down the street one afternoon when they met a kind old man. When the old man asked them about the size of their family, the boy quicklyanswered. “I have as many brothers as I have sisters,” he proudly stated. Not to be left out, the girl added, “I have three times as many brothers as I have sisters.” Can you tell how many boys and girls in total there were in their family? A) 5 B) 6 C) 7 D) 8A farmer was asked how many pigs he had. “Well,” he said, “if I had just as m any more again, plus half as many more, plus another 1.5 times more, I would have three dozen.” How many pigs did he have?A) 6 B) 9 C) 12 D) 15 15% of 80 is 40% ofA) 30B) 55C) 105D) 210It took me 90 minutes to cycle 45 km to the beach. Later I got a ride from the beach tothe park at twice my cycling speed. If the ride to the park took 15 minutes, what distance did I travel from the beach to the park?A) 15 kmB) 30 kmC) 45 kmD) 135 kmTed, Rick, and Sam painted a wall together. Ted painted 80% more ofthe wall than Sam painted. Sam painted 40% less than Rick. Ted painted ? of the amount that Rick painted. A) 102% B) 108% C) 120% D) 140% The greatest integer power of 20 that is a divisor of 5050 isA) 2020B) 2025C) 2050D) 2012529. The ones digit of the sum of all even integers from 2 to 1492 is A) 2B) 4 C) 8 D) 030. The median of 12, 13, 14, 15, 16, and 17isA) 18 B) 223840 C) 514 D) 94031. The average of all positive even integers from 2 to 2018 is A) 1000 B) 1009 C) 1010 D) 1014 32. Pirate Percy has 300 coins in his chest. Of the Spanish coins,20% are gold. If 100 of the coins are gold but not Spanish and 70 of the coins are neither gold nor Spanish, how many Spanish gold coins are in Percy’s chest?A) 20 B) 26 C) 30 D) 3433. When I divided the population of my city by the number of streets in the city, I got a remainder of 18. If the exact quotient on my calculator was 123.06, how many streets are there in my city? A) 60 B) 120 C) 186 D) 30034. What is the greatest number of 3-by-7 rectangles that can be placed inside an 80-by-90 rectangle with no overlapping?A) 312B) 330C) 334D) 34235. How many four-digit whole numbers have four different even digitsand a ones digit greater than its thousands digit?A) 36B) 54C) 60D) 9036. Both arcs AB and AD are quarter circles of radius 5, figure on the right.Arc BCD is a semi-circle of radius 5. What is the area of the region ABCD ? A) 25 B) 10 + 5π C) 50 D) 50 + 5π 37. In the figure on the right, the side-length of the smaller square is 4. The four arcs are four semi-circles. Each side of square ABCD is tangent to one of the semi-circles. The area of ABCD is A) 32 B) 36 C) 48 D) 6438. A million is a large number, a “1” followed by 6 zeros. A googol is a large number, a “1” followed by one hundred zeros. Agoogo lplex is a large number, a “1” followed by a googol of zeros. A googolplexian is a large number, a “1” followed by a googolplex of zeros. A googolplexian isA) 1010 B) 1001010 C) 100101010 D) none of the above 39. If the total number of positive integral divisors of n is 12, what is the greatest possibletotal number of positive integral divisors of n 2? A) 23 B) 24 C) 33 D) 4540. Of all the isosceles triangles whose perimeter is 20 and whose side-lengths are integers, what is the length of the base of the triangle with the largest area? A) 2 B) 5 C) 6 D) 8第3页，共4页第4页，共4页。