2016-2017美国数学大联盟杯赛五年级初赛试题及答案
美国“数学大联盟杯赛” 中国赛区 初赛五年级试卷

2017-2018年度美国“数学大联盟杯赛”(中国赛区)初赛(五年级)(初赛时间:2017年11月26日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论, 我确定我所填写的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
请在装订线内签名表示你同意遵守以上规定。
考前注意事项:1. 本试卷是五年级试卷,请确保和你的参赛年级一致;2. 本试卷共4页(正反面都有试题),请检查是否有空白页,页数是否齐全;3. 请确保你已经拿到以下材料:本试卷(共4页,正反面都有试题)、答题卡、答题卡使用说明、英文词汇手册、 草稿纸。
考试完毕,请务必将英文词汇手册带回家,上面有如何查询初赛成绩、 及如何参加复赛的说明。
其他材料均不能带走,请留在原地。
选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1. The smallest possible sum of two different prime numbers isA) 3B) 4C) 5D) 62. The greatest common factor of two numbers is3. The product of these two numbers mustbe divisible byA) 6 B) 9 C) 12 D) 18 3. The sum of 5 consecutive one-digit integers is at most A) 15 B) 25 C) 35 D) 45 4. How many two-digit multiples of 10 are also multiples of 12?A) 4B) 3C) 2D) 15. I have read exactly13of the total number of chapters in my 120-page book. If each chapter has the same whole number of pages, then the total number of chapters I have left could beA) 16 B) 24 C) 32 D) 50 6. What is the greatest odd factor of 44 × 55 × 66?A) 36 B) 55 C) 35 × 55 D) 36 × 55 7. What is the sum of the factors of the prime number 2017? A) 2016B) 2017C) 2018D) 20198. Lynn ran in 6 times as many races as the number of racesshe won. How many of her 126 races did Lynn not win?A) 21B) 90C) 96D) 1059. The least common multiple of 8 and 12 is the greatest common factor of 120 andA) 80B) 124C) 144D) 18010. January has the greatest possible number of Saturdays when January 1 occurs on any ofthe following days of the week exceptA) Thursday B) Friday C) Saturday D) Sunday 11. The number that is 10% of 1000 is 10 more than 10% ofA) 90B) 100C) 900D) 99012. The sum of 16 fours has the same value as the product of ? fours.A) 2 B) 3 C) 4 D) 16 13. Of the following, which is the sum of two consecutive integers?A) 111 111B) 222 222C) 444 444D) 888 88814. Abe drove for 2 hours at 30 km/hr. and for 3 hours at 50 km/hr. What was Abe’s averagespeed over the 5 hours?A) 35 km/hr.B) 40 km/hr.C) 42 km/hr.D) 45 km/hr.15. My broken watch runs twice as fast as it should. If my watch first broke at 6:15 P.M.,what time was displayed on my watch 65 minutes later?A) 7:20 P.M. B) 7:25 P.M.C) 8:20 P.M. D) 8:25 P.M.16. (2018 × 2017) + (2018 × 1) =A) 20172 B) 20182 C) 20183D) (2018 + 2017)217. A prized bird lays 2, 3, or 4 eggs each day. If the bird laid 17 eggs in 1 week,on at most how many days that week did the bird lay exactly 2 eggs?A) 2B) 3C) 4D) 518. Of the following, which could be the perimeter of a rectangle whoseside-lengths, in cm, are prime numbers?A) 10 cmB) 22 cmC) 34 cmD) 58 cm19. The average of all possible total values of a 4-coin stack of nickels and dimes (containingat least one of each coin) isA) 20¢B) 30¢C) 40¢D) 60¢20. The diameter of Ann’s drum i s 40 cm more than the radius. What is half the circumference of the drum?A) 120π cmB) 80π cmC) 60π cmD) 40π cm21. Of the following, which expression has the greatest number offactors that are multiples of 2018?A) 2018 × 12B) 20182C) 20192D) 20192019第1页,共4页 第2页,共4页22. When the sum of the factors of a prime number is divided by that prime number, theremainder isA) 0 B) 1 C) 2 D) 3 23. What is the sum of the digits of the greatest integer that has a square root less than 100? A) 18B) 36C) 99D) 10024. My favorite number has 6 different factors. If the product of all 6 factors is 123, what isthe sum of the factors of my favorite number?A) 24B) 28C) 32D) 3625. For how many different pairs of unequal positive integers less than 10 is the least commonmultiple of the numbers less than their product?A) 6B) 7C) 8D) 926. Exactly 12 of the students in my class have at least one brother, and 12 have at least onesister. If 13have no siblings, what fraction of the students in my class have at least onebrother and at least one sister?A) 16 B) 15 C) 14 D) 1327. Each day, Sal swims a lap 1 second faster than on the daybefore. If Sal swims a lap in 60 minutes on the 1st day, on what day does he swim a lap in 10% less time than the 1st day?A) 359th B) 360th C) 361st D) 362nd 28. 20172018 × 20172019 = 2017 ? × 20171009A) 1010B) 2010C) 3028D) 403829. Both arcs AB and AD are quarter circles of radius 5, figure on the right.Arc BCD is a semi-circle of radius 5. What is the area of the region ABCD ?A) 25 B) 10 + 5π C) 50D) 50 + 5π30. For every $5 I earn from my job, I save $2. For every $4 I save from my job, I am givenan additional $1 from my parents to add to my savings. How much must I earn in order to have $40 in savings?A) $160B) $120C) $100D) $8031. In the figure on the right, the side-length of the smaller squareis 4. The four arcs are four semi-circles. Each side of square ABCD is tangent to one of the semi-circles. The area of ABCD isA) 32B) 36C) 48D) 6432. A million is a large number, a “1” follo wed by 6 zeros. A googol is a large number, a “1”followed by one hundred zeros. A googolplex is a large number, a “1” followed by a googol of zeros. A googolplexian is a large number, a “1” fo llowed by a googolplex of zeros. A googolplexian isA) 10100 B) 1001010C) 100101010D) None of the above33. An integral triangle is a triangle with positive integral side-lengths and a positive area.Such a triangle can have a perimeter as small as 3. What is the next smallest possible perimeter of an integral triangle?A) 4B) 5C) 6D) 734. 2 liter of 2% fat milk + 3 liter of 3% fat milk = 5 liter of ? fat milkA) 2.5%B) 2.6%C) 5%D) 6%35. One day, a motorist came to a hill that was ten-mile drive up one side and a ten-mile drivedown the other. He drove up the hill at an average speed of 30 miles per hour. How fast will he have to drive down the other side to average 60 miles per hour for the entire 20-mile distance?A) 30 miles per hour B) 60 miles per hour C) 90 miles per hour D) None of the above 36. What is the weight of a fish if it weighs ten pounds plus half its weight?A) 10B) 15C) 20D) 2537. Without using pennies, how many different combinations of coins (nickels, dimes,quarters) will make 30 cents?A) 3B) 4C) 5D) 638. A man once bought a fine suit for which he paid $30 more than14of its price. How much did he pay for the suit? A) $30B) $35C) $40D) $4539. A father is five times as old as his son. In fifteen years he will be only twice as old. Howold is the father at present?A) 40B) 35C) 30D) 2540. It takes 30 minutes to completely fill a tank. If, however, a hole allows13of the water that is entering the tank to escape, how long will it then take to fill the tank?A) 40 B) 45 C) 60 D) 90第3页,共4页第4页,共4页。
五年级美国大联盟应用题专项(含题目翻译答案解析)
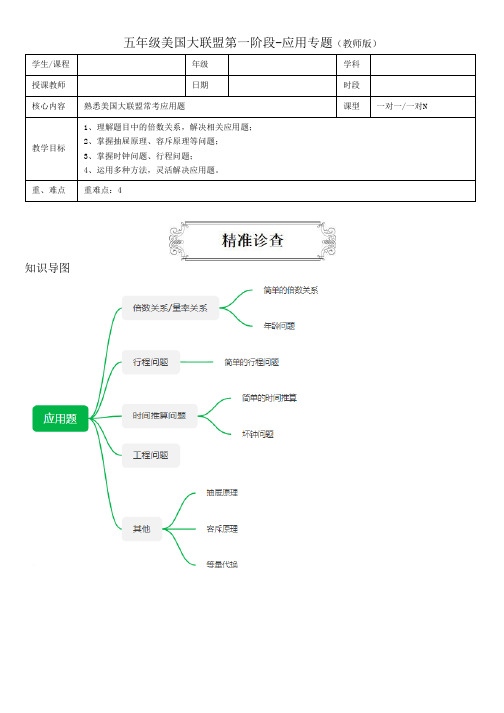
五年级美国大联盟第一阶段-应用专题(教师版)学生/课程年级学科授课教师日期时段核心内容熟悉美国大联盟常考应用题课型一对一/一对N教学目标1、理解题目中的倍数关系,解决相关应用题;2、掌握抽屉原理、容斥原理等问题;3、掌握时钟问题、行程问题;4、运用多种方法,灵活解决应用题。
重、难点重难点:4 知识导图导学一:倍数关系/量率关系知识点讲解1、简单的倍数关系(1)倍数问题小数(一倍数)×倍数=大数大数÷倍数=小数(一倍数)单位1×分率=对应量对应量÷分率=单位1(2)如何判断“一倍数”/“单位1”“的”前“比”后(3)解题方法①画图法:画线段图,用一格表示一倍数②方程法:设一倍数为X例题1.[单选题] [整数、小数复合应用题] [难度:★★★ ] Lynn ran in 6 times as many races as the number of races she won. How many of her 126 races did Lynn not win?A)21 B)90 C)96 D)105【参考答案】D【题目解析】翻译:Lynn参加的比赛是她赢的比赛的6倍,她126场比赛中有()场没有赢。
解析:Lynn参加的比赛是她赢的比赛的6倍,题目中“小数”为“赢的比赛”,求小数用除法:126÷6=21(场),没有赢的比赛:126-21=105(场)故选D。
2.[单选题] [列方程解含有一个量的应用题] [难度:★★★ ] What is the weight of a fish if it weighs ten pounds plus half its weight?A)10 B)15 C)20 D)25【参考答案】C【题目解析】翻译:如果一条鱼的重量是十磅加上它重量的一半,那么它的重量是多少? 解析:方法一:方程法。
解:设鱼的重量为X,则:X=10+X÷2,解得x=20方法二:算术法。
美国数学大联盟杯赛五年级试卷

2018-2019年度美国“数学大联盟杯赛”(中国赛区)初赛(五年级)(初赛时间:2018年11月14日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
如果您同意遵守以上协议请在装订线内签名选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1. A 6 by 6 square has the same area as a 4 by ? rectangle.A) 3 B) 6 C) 8 D) 92.Every prime has exactly ? positive divisors.A) 1 B) 2 C) 3 D) 4 or more3.If I answered 34 out of 40 questions on my math test correctly, I answered ? % of thequestions correctly.A) 75 B) 80 C) 85 D) 904.120 ÷ 3 ÷ 4 × 12 =A) 1 B) 10 C) 12 D) 1205.10 × 20 × 30 × 40 = 24 ×?A) 1000 B) 10 000 C) 100 000 D) 1000 0006.One of my boxes contains 1 pencil and the others each contain 5 pencils. If there are 101pencils in my boxes, how many boxes do I have?A) 19 B) 20 C) 21 D) 227.An electrical company imports 2016 light bulbs. Unfortunately, 25%of those are damaged. How many light bulbs are not damaged?A) 25 B) 504 C) 1512 D) 20168.50 × (16 + 24) is the square ofA) -40 B) -4 C) 4 D) 809.Which of the following numbers has exactly 3 positive divisors?A) 49 B) 56 C) 69 D) 10010.Ten people stand in a line. Counting from the left, Jerry stands at the 5th position.Counting from the right, which position is he at?A) 4 B) 5 C) 6 D) 7 11.On a teamwork project, Jack contributed 2/7 of the total amount of work, Jill contributed1/4 of the work, Pat contributed 1/5 of the work, and Matt contributed the rest. Whocontributed the most toward this project?A) Jack B) Jill C) Pat D) Matt12.Which of the following numbers is a factor of 2016?A) 5 B) 11 C) 48 D) 9913.2 × 4 × 8 × 16 × 32 × 64 =A) 210B) 215C) 221D) 212014.On a game show, Al won four times as much as Bob, and Bob won four times as much asCy. If Al won $1536, how much did Al, Bob, and Cy win together?A) $96 B) $384 C) $1920 D) $201615.The sum of two composites cannot beA) odd B) even C) 11 D) 1716.If a and b are positive integers such that a/b = 5/7, then a + b isA) 12 B) 24 C) 36 D) not able to be determined17.What is the greatest odd factor of the number of hours in all the days of the year 2015?A) 3 B) 365 C) 1095 D) 328518.If the current month is February, what month will it be 1 199 999 months from now?A) January B) February C) March D) April19.Two angles are complementary. One of these angles is 36° less than the other. What is themeasure of the larger angle?A) 36°B) 54°C) 63°D) 72°20.(The square root of 16) + (the cube root of 64) + (the 4th root of 256) =A) 12 B) 24 C) 32 D) 6421.In ∆ABC, m∠A–m∠B = m∠B–m∠C. What is the degree measure of ∠B?A) 30 B) 60 C) 90 D) 12022.For every 3 math books I bought, I bought 2 biology books. I bought 55 books in all. Howmany of those are math books?A) 11 B) 22 C) 33 D) 4423.John wrote a number whose digits consists entirely of 1s. This number was a compositenumber. His number could contain exactly ? 1s.A) 17 B) 19 C) 29 D) 3224.Weird Town uses three types of currencies: Cons, Flegs, and Sels. If 3 Sels = 9 Cons and 2Cons = 4 Flegs, then 5 Sels = ? Flegs.A) 12 B) 24 C) 30 D) 36第1页,共4页25.If the length of a rectangular prism with volume V is doubled while the width and theheight are halved, the volume of the new prism will beA) 4V B) V/2 C) V D) 2V26.Rick and Roy each stands at different ends of a straight road that is 64 m long. They runtoward each other. Rick’s speed is 3 m/s and Roy’s speed is 5 m/s. They will meet in? seconds.A) 1 B) 2 C) 4 D) 827.If the area of a certain circle is 2016, its radius isA) sqrt(2016/π) B) sqrt(4032/π)C) 2016/πD) 1008/π28.In a toy shop, the cost of a Teddy Bear is 200% as much as that of a toy train. The cost ofa toy train is 6/5 the cost of a pack of the wooden blocks. The cost of a pack of woodenblocks is $50. What is the cost, in dollars, of the Teddy Bear?A) 60 B) 100 C) 120 D) 20029.In the sequence 2016, 225, 141, 66, 432, 99, 1458 …, each term after the first term is thesum of the cubes of the digits of the previous term. What is the 100th term of thissequence?A) 153 B) 351 C) 370 D) 37130.What is the sum of all the positive divisors of 210?A) 210 – 1 B) 211 – 1 C) 212 – 1 D) 213 – 131.It takes 4 hours for Mike and Lucy to finish a task. It takes Lucy and Jerry 5 hours tofinish the same task. And it takes 6 hours for Mike and Jerry to finish the same task. Lucy and Jerry first work on the task for 1 hour and 45 minutes. Then Mike takes over the task on his own. How many more hours does it take for Mike to finish the task?A) 3 B) 4 C) 5 D) 632.If you sell a cloth at its current price, you get $40 profit. The total profit you get selling 10clothes at 70% of its current price is equal to the total profit you get selling 20 clothes at $82 per cloth. What is the current price of a cloth?A) 80 B) 100 C) 120 D) 12533.There are 6 identical squares in the figure on the right. The side length of eachsquare is 1. Of all the triangles constructed by connecting three of the 18vertices in the figure, how many of them are triangles whose area is 2 andwhich has at least one vertical or horizontal side?A) 12 B) 16 C) 24 D) 2834.Pick up N numbers from 1 to 2015 inclusively, such that the sum of any three of the Nnumbers is divisible by 24. What is the maximum value of N?A) 83 B) 84 C) 168 D) 252 35.汤姆有一件花了64美金买来的衬衫,他打算以比原价高出25%的价格出售,他会卖出多少钱?A) $16 B) $32 C) $48 D) $8036.满足以下条件的最小整数是多少:“除以3余2,除以5余4,除以7余6。
2016年第十四届”走美杯“小学数学竞赛杭州赛区试卷(五年级初赛答案及解析)

2016年第十四届”走美杯“小学数学竞赛杭州赛区试卷(五年级初赛)-学生用卷一、填空题共5题,共40 分1、计算:(写成小数的形式,精确到小数点后两位)2、角硬币的正面与反面如图所示,拿三个角硬币一起投掷一次,得到两个正面一个反面的概率为。
3、大于的自然数,如果满足所有因数之和等于它自身的倍,则这样的数称为完美数或完全数。
比如,的所有因数为,,,,,就是最小的完美数,是否有无限多个完美数的问题至今仍然是困扰人类的难题之一,研究完美数可以从计算自然数的所有因数之和开始。
那么的所有因数之和为。
4、某大型会议上,要从小张、小赵、小李、小罗、小王五名志愿者中选派四人分别从事翻译、导游、礼仪、司机四项不同工作,若其中小张和小赵只能从事前两项工作,其余三人均能从事这四项工作,则不同的选派方案共有种。
5、将从开始到的连续的自然数相乘,得到,记为(读作的阶乘)用除显然,被整除,得到一个商,再用除这个商,,这样一直用除下去,直到所得的商不能被整除为止。
那么,在这个过程中用整除了次。
二、填空题共5题,共50 分6、如图,已知正方形中,是边的中点,,是与的交点,四边形的面积与正方形的面积的比是。
7、如图所示,将一张纸沿着长边的个中点对折,得到个小长方形,小长方形的长与宽之比与纸相同。
如果设纸的长为厘米,那么,以纸的宽为边长的正方形面积为平方厘米(精确到小数点后一位)。
8、由一些顶点和边构成的图形称为一个图,对一个图用不同颜色给顶点染色,要求具有相同边的两个顶点染不同的颜色。
称为图的点染色,图的点染色通常要研究的问题是完成染色所需要的最少的颜色数,这个数称为图的色数。
如图的图称为彼特森图,彼特森图的色数为。
9、在平面上,用边长为的单位正方形构成正方形网格,顶点都落在单位正方形的顶点(又称为格点)上的简单多边形叫做格点多边形。
最简单的格点多边形是格点三角形,而除去三个顶点之外,内部或边上不含格点的格点三角形称为本原格点三角形,如图所示的本原格点三角形,每一个格点多边形都能够很容易地划分为若干个本原格点三角形。
2017年五年级创新杯初赛真题及详解
【分析】
1 9 11 13 1 8 8 10 8 10 12 8 10 12 14
1 9 11 1 8 8 10 8 10 12 8 10 12
1 9 11 1 8 8 10 8 10 12
1 9 1 8 8 10 8 10
原式= 37037037037 73 1 2703703703701
12. 在 60 米赛跑中,甲到终点时乙还有 10 米、丙还有 20 米,按照这样的速度,乙到终点 时丙还有________米. 【考点】行程 【答案】12 【分析】甲跑 60 米,乙跑 60-10=50 米,丙跑 60-20=40 米 则乙跑完 50 米时,丙可跑 60-20=40 米。 当乙跑完剩下的 10 米时,丙又跑 40÷5=8 米 则还剩 60-(40+8)=12 米
).
A.99
B.81
C.27
D.18
【考点】数字和
【答案】B
【分析】记两位数的数字和为 M,并且进了 k 次位,则可得 2M-9k=M
可得 M=9k(即 M 是 9 的倍数)
M 最大为 18,即两位数为最大 99;
M 最小为 9,即两位数最小为 18;
则差为 99-18=81.
9. 暗箱中有五张分别写 1、2、3、4、5 数字的卡片,从中随机摸出三张,由这三张上的数
和与这三个大学生的岁数和相等,还需要经过( )年.
A.18
B.12
C.9
D.6
【考点】年龄问题
【答案】C
【分析】假设还需要 x 年,则 x 年后年龄和变为 54 5x 72 3x
美国数学大联盟杯赛五年级试卷(2020新教材)

——教学资料参考参考范本——美国数学大联盟杯赛五年级试卷(2020新教材)______年______月______日____________________部门(初赛时间:2018年11月14日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
如果您同意遵守以上协议请在装订线内签名选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1. A 6 by 6 square has the same area as a 4 by ? rectangle.A) 3 B) 6 C) 8 D) 92.Every prime has exactly ? positive divisors.A) 1 B) 2 C) 3 D) 4 or more3.If I answered 34 out of 40 questions on my math testcorrectly, I answered ? % of the questions correctly.A) 75 B) 80 C) 85 D) 904.120 ÷ 3 ÷ 4 × 12 =A) 1 B) 10 C) 12 D) 1205.10 × 20 × 30 × 40 = 24 × ?A) 1000 B) 10 000 C) 100 000 D) 1000 0006.One of my boxes contains 1 pencil and the others each contain 5 pencils. If there are101 pencils in my boxes, how many boxes do I have?A) 19 B) 20 C) 21 D) 227.of those are damaged. How many light bulbs are not damaged?A) 25 B) 504 C) 1512 D) 20xx8.50 × (16 + 24) is the square ofA) -40 B) -4 C) 4 D) 809.Which of the following numbers has exactly 3 positive divisors?A) 49 B) 56 C) 69 D) 10010.Ten people stand in a line. Counting from the left, Jerrystands at the 5th position. Counting from the right, which position is he at?A) 4 B) 5 C) 6 D) 711.On a teamwork project, Jack contributed 2/7 of the totalamount of work, Jill contributed 1/4 of the work, Patcontributed 1/5 of the work, and Matt contributed the rest.第1页,共4页Who contributed the most toward this project?A) Jack B) Jill C) Pat D) Matt12.Which of the following numbers is a factor of 20xx?A) 5 B) 11 C) 48 D) 9913.2 × 4 × 8 × 16 × 32 × 64 =A) 210B) 215C) 221D) 212014.On a game show, Al won four times as much as Bob, and Bobwon four times as much as Cy. If Al won $1536, how much did Al, Bob, and Cy win together?A) $96 B) $384 C) $1920 D) $20xx15. cannot beA) odd B) even C) 11 D) 1716.If a and b are positive integers such that a/b = 5/7, thena +b isA) 12 B) 24 C) 36 D) not able to be determined17.What is the greatest odd factor of the number of hours in all the days of the year 20xx?A) 3 B) 365 C) 1095 D) 328518. If the current month is February, what month will it be 1199 999 months from now?A) January B) February C) March D) April 19. ° less than the other. What is the measure of the larger angle?A) 36°B) 54°C) 63°D) 72°20. (The square root of 16) + (the cube root of 64) + (the 4throot of 256) =A) 12B) 24C) 32D) 6421. In ∆ABC, m ∠A – m ∠B = m ∠B – m ∠C. What is the degreemeasure of ∠B?A) 30B) 60C) 90D) 12022. For every 3 math books I bought, I bought 2 biology books. I bought 55 books in all. How many of those are math books?A) 11 B) 22C) 33D) 4423. ? 1s.A) 17B) 19C) 29D) 3224. Weird Town uses three types of currencies: Cons, Flegs, and Sels. If 3 Sels = 9 Cons and 2 Cons = 4 Flegs, then 5 Sels = ? Flegs.A) 12B) 24 C) 30 D) 3625. If the length of a rectangular prism with volume V isdoubled while the width and the height are halved, the volume of the new prism will beA) 4VB) V /2C) VD) 2V26. Rick and Roy each stands at different ends of a straight road that is 64 m long. They run toward each other. Rick ’s speed is 3 m/s and Roy ’s speed is 5 m/s. They will meet in? seconds.……………线…………………………………………………………… ……………答…………………题………………………………………。
【2020】最新美国数学大联盟杯赛五年级试卷

(初赛时间:2018年11月14日,考试时间90分钟,总分200分)学生诚信协议:考试期间,我确定没有就所涉及的问题或结论,与任何人、用任何方式交流或讨论,我确定以下的答案均为我个人独立完成的成果,否则愿接受本次成绩无效的处罚。
如果您同意遵守以上协议请在装订线内签名选择题:每小题5分,答对加5分,答错不扣分,共200分,答案请填涂在答题卡上。
1. A 6 by 6 square has the same area as a 4 by ? rectangle.A) 3 B) 6 C) 8 D) 92.Every prime has exactly ? positive divisors.A) 1 B) 2 C) 3 D) 4 or more3.If I answered 34 out of 40 questions on my math test correctly, I answered ? % of the questionscorrectly.A) 75 B) 80 C) 85 D) 904.120 ÷ 3 ÷ 4 × 12 =A) 1 B) 10 C) 12 D) 1205.10 × 20 × 30 × 40 = 24 × ?A) 1000 B) 10 000 C) 100 000 D) 1000 0006.One of my boxes contains 1 pencil and the others each contain 5 pencils. If there are 101 pencils inmy boxes, how many boxes do I have?A) 19 B) 20 C) 21 D) 227.of those are damaged. How many light bulbs are not damaged?A) 25 B) 504 C) 1512 D) 20xx8.50 × (16 + 24) is the square ofA) -40 B) -4 C) 4 D) 809.Which of the following numbers has exactly 3 positive divisors?A) 49 B) 56 C) 69 D) 10010.Ten people stand in a line. Counting from the left, Jerry stands at the 5th position. Counting fromthe right, which position is he at?A) 4 B) 5 C) 6 D) 72 / 4第1页,共4页23. ? 1s.4 Flegs, then5 Sels = ? Flegs.。
五年级数学杯赛答案

答案应为:441.0
注:441 为错误答案,保留一位小数概念不清
10. 1 2 解析: 这个游戏的题的策略是奇数性的利用。只要取成 3 号格和 1 号格里的球数 相同即可确保获胜。 所以,要确保获胜,先手必须将 1 号格中的 2 个小球移入 0 号格。后手无论怎么移,都会导致这两格球数不一样,先手只须保持两格一样 即可最后获胜。 11. 2 80
8. 3 解析: 这个图形的外围 5 个顶点,至少需要三种颜色才能做到有公共线段的两点 颜色不同;所以,至少需要三种颜色,用三种颜色涂各点可得如上图,所以,皮 特森图的色数为 3。 9. 36
2
曹老师奥数 15195810017
竞赛真题集
解析;令一个小正方形的面积为 1,则最小本原格点三角形的面积为 0.5 题目中的区域面积为 18,所以,最多可以划分为 18÷0.5=36 个本原格点三角 形 最少可以划分为 5 个本原格点三角形。最少的情况如下图:
4. 36 解析:根据题意分 2 种情况讨论, ①若小张或小赵入选,则有选法 C21C21A33=24; ②若小张、小赵都入选,则有选法 A2 A3 =12, 共有选法 12+24=36 种, 5. 10
25 8 =8, =2,8 2=10 3 解析: 3
曹老师奥数 15195810017
竞赛真题集
杯赛试题集参考答案
1.2016 年第十四届“走进美妙的数学花园”青少年展示交流活动--趣味数学解 题技能展示大赛初赛.................................................2 2. 2015 年第十三届“走进美妙的数学花园”青少年展示交流活动--趣味数学解 题技能展示大赛初赛................................................5 3. 2014 年第十二届“走进美妙的数学花园”青少年展示交流活动--趣味数学解 题技能展示大赛初赛.................................................9 4.第二十二届华罗庚金杯少年数学邀请赛初赛试题(小学高年级组)........10 5. 第二十一届华罗庚金杯少年数学邀请赛初赛试题(小学高年级组).......13 6. 第二十一届华罗庚金杯少年数学邀请赛决赛试题(小学高年级组).......16 7. 第二十届华罗庚金杯少年数学邀请赛初赛试题 (小学高年级组) ..........20 8. 第二十届华罗庚金杯少年数学邀请赛决赛决题 (小学高年级组) ..........24 9. 第十九届华罗庚金杯少年数学邀请赛决赛试题(小学高年级组).........27 10. 2016 年第十四届小学“希望杯”全国数学邀请赛第一试.............. 30 11. 2016 年第十四届小学“希望杯”全国数学邀请赛第二试.............. 33 12. 2015 年第十三届小学“希望杯”全国数学邀请赛第一试.............. 35 13. 2015 年第十三届小学“希望杯”全国数学邀请赛第二试.............. 40 14. 2014 年第十二届小学“希望杯”全国数学邀请赛第一试.............. 42 15. 2014 年第十二届小学“希望杯”全国数学邀请赛第二试.............. 45 16. 2013 年第十一届小学“希望杯”全国数学邀请赛第一试.............. 49 17. 2013 年第十一届小学“希望杯”全国数学邀请赛第二试.............. 46 18. 2016 年“数学花园探秘”科普活动 五年级组初试试卷 A............. 49 19. 2016 年“数学花园探秘”科普活动 五年级组决试试卷 A............. 54 19. 2016 年书人教育春季五年级数学期中测试卷.........................54 20. 2015 年书人教育春季五年级数学期中测试卷.........................59 21. 2014 年书人教育春季五年级数学期中测试卷.........................64 22. 2014 年书人教育春季五年级数学期末测试卷.........................68 23. 2016 年书人教育春季五年级数学期末测试卷.........................73 24. 2015 年书人教育春季五年级数学期末测试卷.........................76 25. 参考答案
