运筹学英文版北信科作业
运筹学学报英文版返修流程

运筹学学报英文版返修流程Here's a sample of the revision process for the English version of an Operations Research journal, written in an informal and conversational style while maintaining diversity in language usage and avoiding any connecting or transitional phrases:For the English revision of your operations research paper, it's essential to keep the focus on clarity and precision. The editor's feedback will likely highlight areas where you need to refine your writing. Start by tackling the bigger issues first, like reorganizing sections or adding missing details.Don't be afraid to make significant changes. Operations research thrives on logic and precision, so make sure your revisions reflect that. If the editor suggests a different methodology, consider it carefully and adjust your paper accordingly.The language should be precise but accessible. Avoid jargon or overly technical language unless it's absolutely necessary. Remember, your goal is to communicate your ideas effectively, not to impress with fancy words.When you're done with the big revisions, don't forget the smaller details. Check for grammar, spelling, and punctuation errors. Even the smallest mistake.。
运筹学作业d文档

运筹学作业d⽂档注:没有选项的题是判断题,每⼀章的前⼏道都是判断题Chapter 11. Managers do not need to know the mathematical theory behind the techniques of management science in order to lead management science teams.2. Management scientists are responsible for making the managerial decisions for an organization.3. Once management makes its decisions, the management science team typically continues to work to implement the new plan.4. At the break-even point, the fixed cost equals the variable cost.5. Sensitivity analysis is used to check the effect on the recommendations of a model if the estimates turn out to be wrong.6. Enlightened future managers do not need to know which of the following?A. How the models of management science are solved.B. When management science can and cannot be applied.C. How to apply the major techniques of management science.D. How to interpret the results of a management science study.E. None of the above.7. Which of the following is not a component of a mathematical model for decision making?A. Decision variables.B. A spreadsheet.C. Constraints.D. Parameters.E. All of the above.8. Which of the following is not a step taken in a typical management science study?A. Define the problem and gather data.B. Formulate a model.C. Apply the model and develop recommendations.D. Help to implement the recommendation.E. All of the above are typical steps in a management science study.9. Which of the following is true at the break-even point?A. The fixed cost equals the variable cost.B. The production quantity equals the sales forecast.C. The company will neither make nor lose money on the product.D. The profit equals the cost.E. None of the above.10. A constraint in a mathematical model isA. a variable representing the decision to be made.B. an inequality or equation that restricts the values of the variables.C. a measure of the performance of the model.D. the sales forecast.E. none of the above.Chapter 21. Linear programming problems may have only one goal or objective specified.2. A feasible solution is one that satisfies at least one of the constraints of a linear programming problem.3. The cell containing the measure of performance is referred to as a changing cell.4. A linear programming problem can have only one optimal solution.5. When solving a maximization problem graphically, it is generally the goal to move the objective function line in, toward the origin, as far as possible.6. In a linear programming spreadsheet model, the output cells can typically be expressed as a SUMPRODUCT function.7. Changing only the right-hand side of a constraint creates parallel constraint boundary lines.8. The Assume Nonnegative option assures that the target cell will remain nonnegative.9. Which of the following is a component of a linear programming model?A. Constraints.B. Decision variables.C. Parameters.D. An objective.E. All of the above.10. Which of the following are not types of cells in a linear programming spreadsheet model?A. Changing cellsB. Target cellC. Output cellsD. Input cellsE. Data cells11. For the products x and y, which of the following could be a linear programming objective function?A. C = x + 2y.B. C = x+ 2xy.C. C = x - 2(y-squared).D. C = x + 2x/y.E. All of the above.12. Which of the following is not a step in the graphical method:A. Draw the constraint boundary line for each functional constraint.B. Find the feasible region.C. Determine the slope of one objective function line.D. Find the optimal solution using a straight-edge.E. All of the above are steps in the graphical method.13. Given the following 2 constraints, which solution is a feasible solution for a maximization problem?A. (X1 , X2 ) = (1, 5).B. (X1 , X2 ) = (4, 1).C. (X1 , X2 ) = (4, 0).D. (X1 , X2 ) = (2, 1).E. (X1 , X2 ) = (2, 4).14. What is the cost of the optimal solution for the following problem?A. 0.B. 3.C. 15.D. 18.E. 21.15. A local bagel shop produces bagels (B) and croissants (C). Each bagel requires 6 ounces of flour, 1 gram of yeast, and 2 tablespoons of sugar. A croissant requires 3 ounces of flour, 1 gram of yeast, and 4 tablespoons of sugar. The company has 6,600 ounces of flour, 1,400 grams of yeast, and 4,800 tablespoons of sugar available for today's baking. Bagel profits are 20 cents each and croissant profits are 30 cents each. What is the objective function?A. 2B + 4C <= 4,800.B. (B, C) = (0, 1400).C. P = 0.2B + 0.3C.D. $340.E. None of the above.Chapter 31. There is only one correct way to set up a spreadsheet model.2. In the Everglade Golden Years Company problem, the long-term loan had a lower interest rate than the short-term loan.3. When sketching out a spreadsheet, all of the equations should be entered in the sketch.4. Data should be repeated on the spreadsheet wherever it is needed.5. Numbers should be entered directly into formulas.6. Range names can make equations and the Solver dialogue box easier to read and interpret.7. Shading of cells in the spreadsheet should be avoided.8. The toggle alternates between showing equations and showing values in the cells of the spreadsheet.9. Powerful Excel functions should be used to keep the spreadsheet as concise as possible.10. Using absolute and relative references appropriately makes it easy to expand a model to full-size.11. Which of the following is not a major step in the process of modeling with spreadsheets?A. PlanB. BuildC. TestD. AnalyzeE. All are major steps in the process of modeling with spreadsheets.12. Which of the following are useful steps in the planning stage?A. Visualize where you want to finishB. Do some calculations by handC. Sketch out a spreadsheetD. Sensitivity analysisE. All are useful steps in the planning stage.13. Each constraint should be entered into how many cells on the spreadsheet?A. 1B. 2C. 3D. 4E. 514. Which of the following is not useful for debugging a spreadsheet?A. The auditing toolbar.B. The toggle.C. Trying different values in the changing cells for which you know the solution.D. A and C only.E. All are useful for debugging a spreadsheet.15. Which element of the spreadsheet model should be entered first?A. The data.B. The output cells.C. The target cell.D. The changing cells.E. None of the above.Chapter 41. When formulating a linear programming model on a spreadsheet, the constraints are located in the data cells.2. A mathematical model will be an approximation of the real problem.3. Linear programming must have integer solutions.4. Strict inequalities (i.e., < or >) are permitted in linear programming formulations.5. Once a linear programming problem has been formulated, it is common to make major adjustments to it.6. Resource-allocation problems have constraints for each limited resource.7. A resource constraint has a >= sign in a linear programming model.8. Distribution-network problems typically have mostly <= constraints.9. Which of the following is not a category of linear programming problems?A. Resource-allocation problems.B. Cost-benefit-tradeoff problems.C. Distribution-network problems.D. B and C.E. All of the above are categories of linear programming problems.10. A linear programming model does not contain which of the following components?A. Data.B. Decisions.C. Constraints.D. Measure of performance.E. A spreadsheet.11. Which of the following may not be in a linear programming formulation?A. <=.B. >.C. =.D. A. and C. only.E. All of the above.12. Distribution-network problems have the following type of constraints:A. >=.B. <=.C. >.D. <.E. None of the above.13. Resource-allocation problems typically have which of the following type of constraints:A. >=.B. <=.C. =.D. None of the above.E. All of the above.14. Cost-benefit tradeoff problems typically have which of the following type of constraints:A. >=.B. <=.C. =.D. None of the above.E. All of the above.15. Mixed problems may not have which of the following type of constraints:A. >=.B. <=.C. =.D. All of the above.E. None of the above.Chapter 61. Transportation problems are concerned with distributing commodities from sources to destinations in such a way as to maximize the total amount shipped.2. Transportation problems always have integer solutions if the supplies and demands are all integer.3. The Hungarian Method is an algorithm used to solve assignment problems.4. When demand and supply are not equal in a transportation problem then the problem can be reformulated and solved.5. It is possible to adjust the transportation simplex method to maximize profit instead of minimize cost.6. Which of the following is needed to use the transportation model?A. Capacity of the sources.B. Demand of the destinations.C. Unit shipping costs.D. All of the above.E. None of the above.7. Which of the following is not an assumption or requirement of a transportation problem?A. I and IVB. II and IIIC. I, II and IVD. I and IIIE. I, II, III, and IV8. Which of the following can be modeled as variants of the standard transportation problem?A. The sum of the supplies exceeds the sum of demands.B. A destination has a minimum and maximum demand.C. Certain source-destination combinations cannot be used for distributing units.D. A. and B. only.E. All can be modeled as a variation of the transportation problem.9. An assignment problem:A. will always have an integer solution.B. has all supplies and demands equal to 0.C. always has the demand greater than the supply.D. All of the above.E. None of the above.10. Which of the following is an assumption of assignment problems?A. The number of assignees and the number of tasks are the sameB. The objective is to minimize the number of assignments not made.C. Each task is to be performed by exactly one assignee.D. A. and C. only.E. None of the aboveChapter 71. Each node in a minimum cost flow problem where the net amount of flow generated is a fixed positive number is a supply node.2. If the SUMIF function is used in a network optimization models, it will be nonlinear.3. In a maximum flow problem, the source and sink do not have fixed supplies and demands.4. A shortest path problem may have multiple destinations.5. The number of links in a spanning tree is always the same as the number of nodes.6. The network simplex is a streamlined version of the simplex method.7. Which of the following is not a special type of linear programming problem?A. I and IV.B. I, II, and III.C. II, III, and IV.D. IV only.E. None of the above.8. Which of the following will have positive net outflow in a minimum cost flow problem?A. Supply nodes.B. Transshipment nodes.C. Demand nodes.D. All of the above.E. None of the above.9. Which of the following is not an application of a shortest path problem?A. I and II onlyB. I, II, and III only.C. II onlyD. I, II, III, and IVE. I, III, and IV only.10. If there are 8 nodes in a minimum spanning tree problem then how many links will there be in the solution?A. 6.B. 7.C. 8.D. We cannot tell how many links there will be until it has been solved.E. The total cost will be 7.Chapter 81. In an Activity-On-Arc project network, the nodes are used to separate an activity from each of its immediate predecessors.2. If two paths are tied for the longest duration, the one with the most activities would be considered to be the critical path.3. The slack of an activity is the difference between the latest finish and the latest start times.4. When calculating the probability that a project will finish by a certain time, the approximation that is obtained is usually higher than the true probability.5. The latest finish time for an activity is:A. based on the length of the critical path.B. determined by the maximum of the earliest finish times of its immediate predecessors.C. determined by the maximum of the earliest finish times of its immediate successors.D. the same as the latest start time of its immediate predecessor.E. None of the above.6. Activity C has an early start time of 7, an early finish time of 12, a latest start time of 13, and a latest finish time of 18. Its slack is:A. 0.B. 1.C. 4.D. 6.E. 9.7. Which of the following is a benefit of PERT/CPM?A. It provides an estimate of how long a project will take.B. It allows an activity to overlap with its immediate predecessors.C. It addresses the important issue of how to allocate limited resources.D. A. and C. only.E. All of the above.8. An activity has an optimistic time estimate of four days, a most likely time estimate of eight days, and a pessimistic time estimate of fifteen days. The expected duration of this activity is:A. 7.0 days.B. 7.5 days.C. 8.0 days.D. 8.5 days.E. 10.0 days.9. Which of the following is not a way of crashing an activity?A. Using overtime.B. Hiring more workers.C. Using specialized equipment.D. A. and C.E. All of the above are ways of crashing an activity.10. PERT/Cost does not:A. find the penalty costs if a project is not completed on time.B. compare the actual budget with the planned budget.C. show management where to focus attention during the project.D. A. and B. only.E. B. and C. only.Chapter 91. In an integer programming problem, all of the decision variables are not necessarily required to be integer values.2. Solving the LP relaxation of an integer programming problem and rounding the solution will always find the optimal solution.3. Binary integer programming problems are those where all the decision variables are restricted to integer values.4. Variables whose only possible values are 0 and 1 are called binary variables.5. A problems where all the variables are binary variables is called a mixed BIP problem.6. If choosing one alternative from a group excludes choosing all of the others then these alternatives are called complimentary.7. The constraint x1 +x2 +x3 <= 1 in a BIP represents mutually exclusive alternatives.8. Solving the LP relaxation of an integer programming problem and rounding the solution will find a solution that may not be:A. feasible.B. optimal.C. integer.D. A. and B.E. All of the above.9. Binary integer programming problems can answer which types of questions?A. How much of a product should be produced?B. Should an investment be made?C. Should a plant be located at a particular location?D. All of the above.E. B. and C. only.10. Binary variables can have the following values:A. 0.B. 1.C. any integer value.D. A. and B. only.E. All of the above.11. In a BIP problem with 3 mutually exclusive alternatives, A, B, and C , the following constraint needs to be added to the formulation if two alternatives must be chosen:A. A + B + C <= 2.B. A + B + C = 2.C. A - B - C <= 2.D. A + B + C <= 1.E. None of the above.12. In a BIP problem, 1 corresponds to a yes decision and 0 to a no decision. If project S can be undertaken only if project T is also undertaken then the following constraint needs to be added to the formulation:A. S + T <= 1.B. S + T = 1.C. S <= T.D. T <= S.E. None of the above.13. In a BIP problem, 1 corresponds to a yes decision and 0 to a no decision. If there are 3 projects under consideration (A, B, and C) and at most 2 can be chosen then the following constraint needs to be added to the formulation:A. A + B + C <= 3.B. A + B + C <= 2.C. A + B + C >= 2.D. A + B + C = 2.E. None of the above.14. Auxiliary binary variables can be used to deal with:A. set-up costs for initiating production.B. mutually exclusive products.C. either-or constraints.D. All of the above.E. None of the above.Chapter 101. If the slope of a curve on a profit graph never increases but sometimes decreases as the level of the activity increases, then it is said to have increasing marginal returns.2. The Solver Table can be used to try a variety of starting points in a nonlinear programming problem.3. Separable programming requires that the objective function be piecewise linear.4. The Evolutionary Solver uses an algorithm that is sometimes called a genetic algorithm.5. Evolutionary Solver is a good choice for problems with many constraints.6. The Evolutionary Solver requires that the constraints all be linear.7. Problems with increasing marginal returns are generally easier for Solver to solve than problems with decreasing marginal returns.8. A nonlinear programming problem will have how many local maxima?A. 0B. 1C. 2D. 3E. It can have any number of local maxima.9. A linear function may not contain which of the following?A. A term that contains a single variable with an exponent of 1.B. A term that contains a single variable with an exponent of 2.C. A term that is a constant times the product of two variables.D. B. and C. only.E. All of the above.10. Which of the following Excel functions are linear (assuming changing cells are in C1:C6 and data cells are in D1:D6):A. I only.B. I and II.C. I and III.D. II and IV.E. III and IV.11. Which of the following Excel functions are linear (assuming changing cells are in C1:C6 and data cells are in D1:D6):A. I only.B. I and II.C. II and III.D. I and IV.E. IV only.12. Which of the following is an example of a nonlinear function?A. P= 5 X1+ 7 X2 - 2 (X2-squared).B. P= 8 X1 - 4 X2.C. P= X1 + 6 X2 + 3 X1 X2.D. A. and C. only.E. All of the above.13. The requirement that each term in the objective function only contains a single variable is referred to as:A. the proportionality assumption.B. the divisibility assumption.C. the additivity assumption.D. a nonlinear function.E. None of the above.Chapter 111. The overall objective for a goal programming problem is to determine the most important objective in the problem.2. Goal programming provides two alternative ways of formulating problems with multiple goals: preemptive and weighted goal programming.3. Preemptive goal programming is an appropriate technique when all of the goals are fairly equal in importance.4. In preemptive goal programming it is assumed that there is a distinct order of importance for all goals, and that no goals are of equal importance.5. Preemptive goal programming involves solving a single linear programming model.6. Weighted goal programming involves solving a single linear programming model.7. Goal programming can handle problems with how many different objectives or goals?A. 1.B. 2.C. 3.D. 4.E. Any number of objectives or goals.8. Which of the following are included as changing cells in a goal programming formulation?A. The levels of the various activities.B. The amount over each goal.C. The amount under each goal.D. B. and C. only.E. All of the above are changing cells.9. In weighted goal programming the objective is toA. Maximize profit.B. Minimize cost.C. Achieve the most important goal.D. Minimize a weighted sum of deviations from the various goals.E. Minimize the amount under each goal.10. In preemptive goal programming, the most important thing is toA. Achieve the most important goal.B. Come close to achieving all the goals.C. Ignore the least important goal.D. A. and C.E. All of the above.Chapter 121. Prior probabilities refer to the relative likelihood of past events.2. Bayes' decision rule says to choose the alternative with the largest possible payoff.3. The EVPI indicates how much the payoff will be with perfect information.4. A risk seeker has an increasing marginal utility for money.5. The exponential utility function assumes a variable aversion to risk.6. The maximax criterion is appropriate for the eternal optimist.7. The expected payoff is the payoff that is most likely to occur.8. In a decision tree, the expected payoff of a particular event node is equal to the SUMPRODUCT of the probabilities and expected payoffs of each branch.9. Sensitivity analysis of a decision tree requires the use of Solver Table.10. If C > EVPI then it is not worthwhile to obtain more information.11. Which of the following statements is correct when making decisions?A. The sum of the state of nature probabilities must be 1.B. Every probability must be greater than or equal to 0.C. All probabilities are assumed to be equal.D. A. and B. only.E. All of the above.12. Given the following information what is the maximum likelihood strategy?A. A.B. B.C. C.D. D.E. E.13. Given the following information what is the Bayes' decision rule strategy?A. A.B. B.C. C.D. D.E. E.14. Given the following information what is the expected value of perfect information?A. 4.5.B. 9.C. 40.5.D. 49.5.E. 60.15. Which of the following can be used to do sensitivity analysis with decision trees?A. Trial and error.B. A Data Table.C. SensIt.D. A. and C.E. All of the above.答案:chapter 1Chapter 1 ABABA ABECBChapter 2 ABBBB AABED AEDDCChapter 3 BABBB ABABA EDCEAChapter 4 BABBA ABBEE BEBAEChapter 6 BAAAA DBEADChapter 7 ABABB BDACBChapter 8 ABBAE DADEAChapter 9 ABBAB BADED BCDEChapter 10 BABAB BBEDC EDCChapter 11 BABBB AEEDAChapter 12 BBBAB ABABA DCCBE。
运筹学(英文版)

运筹学(英文版)运筹学B(双语)复习纲要I 概念汇总1)运筹学模型的三要素2)LP标准形式3)(非)基变量(入基变量、出基变量)4)基解、基可行解、解基逆矩阵5)退化6)人工变量法、两阶段法7)LP解的四种情况8)LP对偶问题的形式及最优解9)LP对偶问题的经济解释10)运输表格及表上作业法((非)基变量、入基变量、出基变量)11)网络的基本概念(点、边、权、有向边、链、道路、圈、回路、树、生成树、最小生成树、连通图、割)12)目标规划的含义(模型、偏差变量)13)整数规划模型(分支定界法、割平面法的思路)II 方法汇总1)LP问题图上求解法2)单纯形法3)对偶单纯形法4)运输问题表上作业法(三大步骤)5)求最小生成树6)求最短路问题7)求最大流问题8)目标规划的图解法9)中国邮路问题III题型1)多选题:20分左右2)判断题:10分左右3)简答题:30分左右4)计算题:50分左右《运筹学B》双语课程词汇表Chapter 1 What is Operations Research?Operations Research 运筹学Mathematic model 数学模型decision alternative 决策选择decision variable 决策变量restriction,constraint 约束条件objective criterion 目标准则objective function 目标函数linear programming 线性规划integer programming 整数规划dynamic programming 动态规划network programming 网络规划nonlinear programming 非线性规划algorithm 算法iteration 迭代Chapter 2 Introduction to Linear Programming Graphical solution 图解法Graphical sensitivity analysis 图上灵敏度分析nonnegativity restrictions 非负约束条件feasible solution 可行解optimal feasible solution 最优可行解coefficient 系数denominator 分母infeasible 不可行unit worth 单位价值Chapter 3 The Simplex Methodsolution space 解空间algebraic solution 代数解graphical solution 几何解optimal solution 最优解equation 方程corner point 顶点basic variable 基变量nonbasic variable 非基变量basic solution 基解The Simplex Method 单纯形法iterative 迭代的origin 原点leaving variable 出基变量entering variable 入基变量ratio 比率Gauss-Jordan row operation 高斯-约当行变换pivot column 主列pivot row 主行pivot element 主元素artificial variable 人工变量M-Method 大M方法Two-Phase Method 两阶段方法penalty 罚数degeneracy 退化degenerate 退化的alternative optima 多重最优解infinity 无穷unbounded 无界的pseudo-optimal solution 伪解Chapter 4 Duality and Sensitivity Analysis dual problem 对偶问题primal problem 原问题matrix 矩阵vector 向量identity matrix 单位矩阵verify 证明dual simplex method 对偶单纯形法generalized simplex method 广义单纯形法Chapter 5 Transportation Model and Its Variants Transportation Model 运输模型nontraditional Transportation Model 非典型运输模型The Transportation Algorithm 运输算法source 出发地destination 目的地node 节点arc 边,弧transportation tableau 运输表格balanced 平衡的Northwest-Corner Method 西北角法Least-Cost Method 最小费用法V ogel Approximation Method 沃格尔法The Assignment Model 指派模型Hungarian Method 匈牙利方法Chapter 6 Network Modelsnetwork 网络Minimal Spanning Algorithm 最小生成树算法Shortest-Route Algorithm 最短路算法path 链connected network 连通网络cycle 回路spanning tree 生成树maximal flow 最大流residue network 剩余网络breakthrough path 关键路线Chapter 8 Goal Programminggoal programming 目标规划deviational variable 偏差变量Chapter 9 Integer Linear ProgrammingInteger Linear Programming 整数线性规划integer variables 整数变量Cutting-Plane Algorithm 割平面法B&B Algorithm 分支定界法。
运筹学英文版、优化单纯形法
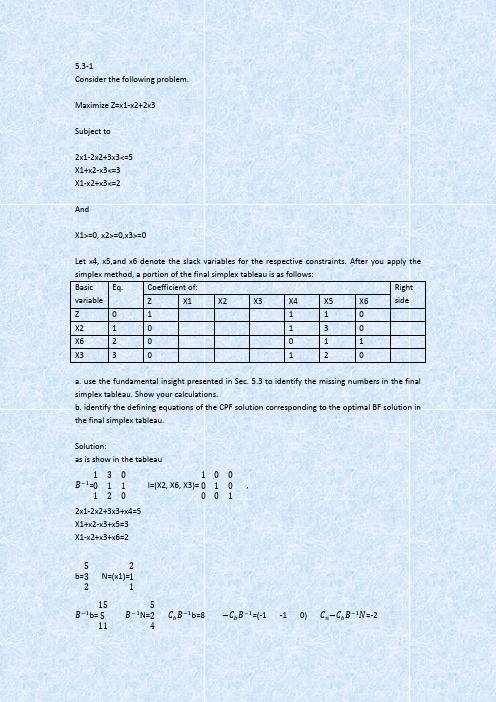
5.3-1Consider the following problem.Maximize Z=x1-x2+2x3Subject to2x1-2x2+3x3<=5X1+x2-x3<=3X1-x2+x3<=2AndX1>=0, x2>=0,x3>=0Let x4, x5,and x6 denote the slack variables for the respective constraints. After you apply the simplex method, a portion of the final simplex tableau is as follows:Basic variable Eq. Coefficient of: Rightside Z X1 X2 X3 X4 X5 X6Z 0 1 1 1 0X2 1 0 1 3 0X6 2 0 0 1 1X3 3 0 1 2 0a. use the fundamental insight presented in Sec. 5.3 to identify the missing numbers in the final simplex tableau. Show your calculations.b. identify the defining equations of the CPF solution corresponding to the optimal BF solution in the final simplex tableau.Solution:as is show in the tableau=I=(X2, X6, X3)=,2x1-2x2+3x3+x4=5X1+x2-x3+x5=3X1-x2+x3+x6=2b=N=(x1)=b=N=b=8 =(-1 -1 0) =-2The simplex tableau:c 1 -1 2 0 0 0cb xb b X1 X2 X3 X4 X5 X6 min-1 X2 14 5 1 0 1 3 00 X6 5 2 0 0 0 1 12 X3 114 0 1 1 2 0z 8 -2 0 0 -1 -1 05.3-2Consider the following problem.Maximize Z=4x1+3x2+x3+2x4,Subject to4x1+2x2+x3+x4<=53x1+x2+2x3+x4<=4AndX1>=0, X2>=0, X3>=0, X4>=0Let x5 and x6 denote the slack variable for the respective constraints. After you apply the simplex method, a portion of the final simplex tableau is as follows:Basic variable Eq. Coefficient of: Rightside Z X1 X2 X3 X4 X5 X6Z 0 1 1 1X2 1 0 1 -1X4 2 0 -1 2a. use the fundamental insight presented in Sec.5.3 to identity the missing number in the final simplex tableau. Show your calculations.b. identify the defining equations of the CPF solution corresponding to the optimal BF solution in the final simplex tableau.Solution:As is show in the tableau:=I =(X2,X4)=,4x1+2x2+x3+x4+x5=53x1+x2+2x3+x4+x6=4b=N=(x1,x3)=b=N==-3 -2The simplex tableau:c 4 3 1 2 0 0cb xb b X1 X2 X3 X4 X5 X6 min 3 X2 1 1 1 -1 0 1 -12 X43 2 0 3 1 -1 2z 9 -3 0 -2 0 -1 -1。
Solutions of assignment 1

Rs R Vin s Vin . R1 Rs R1
The current flowing through the circuit is nearly constant, therefore the voltage across the sensor resistor is nearly proportional to the resistance of the sensor resistor. A good linearity is guaranteed. 3. How to minimize stray capacitance? A: Try to minimize the separation(distance) between the sensor element and the rest of the circuit. 4. Why the so‐called 4‐wire resistance approach can minimize the measurement error in resistance measurement? In 4‐wire approach, current is passed through the leads and the through the sensor element. A second pair of wires is independently attached to the sensor leads, a voltage gauge (with large impedance) is connected, so that the voltage gauge does not draw significant current. So it simply measures the voltage drop across the sensor element alone. 5. Why does the low‐pass filter allow low‐frequency signals through but prevent high‐frequency signals? A: In a low‐pass filter circuit, the impedance of the capacitor decreases at high frequency, so the output decreases as the input frequency increases.
运筹学英文版作业

4.3-4 解:Max. x x x Z 321634++=S.t. 30334321=+++xx x x (1)403225321=+++x x x x (2)And 0≥x i, for i=1,2,3,4,5Initialization:0,00321===x xx ,; so,40,3054==x xThe basic solution (0,0,030,40) is not a optimal solution.Interation 1 : Step1: x x x Z 321634++=, 6>4>3, so, the entering basic variable is x 2Step2:0021==x x ,340340,403min10330,3033355333443≤⇒-==+←≤⇒-==+x x x x x x x x x xso, the leaving basic variable isx 4Step3:)2(10)1(603131)0(602254214321421=+-+-=+++=+-+x x x x x x x x x x x Z The BF solution is (0,0,10,0,10), z=60 Interation 2 : Step1: 6022421+-+-=x x x Z , and x 2is the entering basic variable.0,41=xxmin)1010(10)303110(10312255222332←≤⇒+==+-≤⇒-==+x x x x x x x x x x So, the leaving basic variable is x 5Step2:)2(103131)1(320313254)0(7043215431541=+++=-++=+++x x x x x x x x x x x Z So, the BF solution is (0,10,20/3,0,0), Z=704.4-3 解:(a)图略;x x Z 212+=;CPF: (0,0),(25,0),(0,40),(20,20); Optimal solution is (20,20)(c) Max. 0221=--x x Z S.t.100440421321=++=++x x x x x xInteration 1 :x x Z 212+=, and x 1is the entering basic variable, 02=xmin)254100(1004)4040(401144111331→≤⇒-==+≤⇒-==+x x x x x x x x x xSo, the leaving basic variable isx 425414115414350212142143242=++=-+=+-x x x x x x x x Z The BF solution is (25,0,15,0), z=50 Interation 2:x 2is the entering basic variable,04=x)1004125(,2541min)204315(15432212122332≤⇒-==+←≤⇒-==+x x x x xx x x x x , So, the leaving basic variable isx 320313120313460313243143243=+-=-+=++x x x x x x x x Z The BF solution is (20,20,0,0), Z=60.The BF solution is (20,20,0,0), Z=60. 4.4-5解:(a)Max.8023402260243342632153214321321=+++=+++=+++++=x x xx x x x x x x x x x x x ZInteration 1 :x 2is the entering basic variable, 0,31=xx)380380(803)4040(40min)15460(604226622255222442≤⇒-==+≤⇒-==+←≤⇒-==+x x x x x x x x x x x x x x xSo, the leaving basic variable isx 435432145254123451541214360643154314321431=+-+-=+-+=+++=+-+x x x x xx x x x x x x x x x ZInteration 2 :x 3is the entering basic variable, 0,41=xx)702135(3521min)3502325(2523)302115(1521236633355333232≤⇒-==+←≤⇒-==+≤⇒-==+x x x xx x x x x x x x x x xSo, the leaving basic variable isx 538031323535032616532031313132303265611654154315421541=+---=+-+=-++=+++x x x x x x x x x x x x x x x Z The BF solution is (0,20/3,50/3,0,0,80/3), Z=230/3.(b)4.6-1 解: (a)图略;1,221==xx ;Z=7;(b) Big M method:342032421321421=++=++=+--x x x x x x x x x M Z234.6-7 解:(a) Big M method:50 422020 3526321543216532 1=+++=+-+-=++---xxxx xxxxxxxxxx MMZOptimal solution : (0,0,50,0,0,0), Z=150 (c) Phase 1:(d)(g) The basic solution of the two methods coincide. They are artificial basic solutions for the revised problem until both artificial variables x 5 and x 6 are driven out of the basis, which in the two phase method is the end of phase 1.4.7-3解:(a) 图略;Optimal value of Z : 38; Optimal solution is (8,3)(b) Changing resource 1 to 17 units (1721=x ), cause Z to increase to Z=4*(17/2)+2*(17/6)=119/3, so 3/51*==∆y Z ; Increasing resource 2 to 18 units (18321=+x x ) increase Z to Z=4*(8)+2*(10/3)=116/3, so 3/22*==∆y Z . since constraint 3 is not binding, 03*=y(c) To increase Z by 15, we would need to increase resource 1 by91535151*==y,(Solving the LP with resource 1 set to 16+9=25 conforms that 83*=Z )9.1-3(a )x x x x MinZ 43218.29.27.23+++=S.tThe initial simplex tableau:)4,3,2,1(0454343214231=≥≤+≤+=+=+i x x x x x x x i xx10.3-4Length of shortest path=16Length of shortest path=1。
运筹学Chapter 12 Nonlinear Programming 28May2015

2
Chapter 12 Nonlinear Programming
Convexity C i test for f a function f i of f a single i l variable: i bl Consider any function of a single variable f(x) that possesses a second d derivative d i i at all ll possible ibl values l of x. Then f(x) is 1 convex if and 1. d only l if d2f(x)/dx d 2 0 for f all ll possible ibl values of x; 2 strictly 2. i l convex if and d only l if d2f(x)/dx d 2 > 0 for f all ll possible values of x; 3 concave if and 3. d only l if d2f(x)/dx d 2 0 for f all ll possible values of x; 4 strictly 4. i l concave if and d only l if d2f(x)/dx d 2 < 0 for f all ll possible values o Applications
In Fig.12.1, i 12 1 where h p(x) is i the h price i required i d in i order d to be b able bl to sell x units. The firm’s profit from producing and selling x units of the product then is the sales revenue xp(x) minus the production and distribution costs. Therefore, if the unit cost for producing and distributing the product is fixed at c , the firm’s profit from producing and selling x units is given by the nonlinear function
动态规划(运筹学英文版课后作业答案)
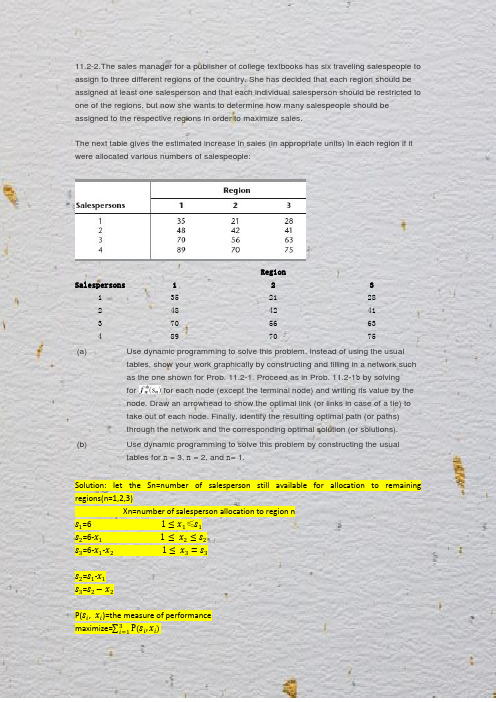
11.2-2.The sales manager for a publisher of college textbooks has six traveling salespeople to assign to three different regions of the country. She has decided that each region should be assigned at least one salesperson and that each individual salesperson should be restricted to one of the regions, but now she wants to determine how many salespeople should be assigned to the respective regions in order to maximize sales.The next table gives the estimated increase in sales (in appropriate units) in each region if it were allocated various numbers of salespeople:Region Salespersons1 2 3 1 35 21 28 2 48 42 41 3 70 56 63 4 897075(a)Use dynamic programming to solve this problem. Instead of using the usual tables, show your work graphically by constructing and filling in a network such as the one shown for Prob. 11.2-1. Proceed as in Prob. 11.2-1b by solving forfor each node (except the terminal node) and writing its value by thenode. Draw an arrowhead to show the optimal link (or links in case of a tie) to take out of each node. Finally, identify the resulting optimal path (or paths) through the network and the corresponding optimal solution (or solutions).(b) Use dynamic programming to solve this problem by constructing the usual tables for n = 3, n = 2, and n = 1.Solution: let the Sn=number of salesperson still available for allocation to remaining regions(n=1,2,3)Xn=number of salesperson allocation to region n s 1=6 1≤x 1≤s 1 s 2=6-x 1 1≤ x 2≤s 2 s 3=6-x 1-x 2 1≤ x 3=s 3s 2=s 1-x 1 s 3=s 2−x 2P(s i , x i )=the measure of performance maximize=∑P(s i ,x i )3i=1f n∗s n=max{ P(s n,x n)+ f n+1∗s n+1}f4∗s4=0S3F3X3128 1241 2363 3475 4f2s2 x21234f2x2 249491 36270702 484838484 1 or 3 59610597981052s1 x11234f1x1 6140132140138140 1 or 3So x1=1 , x2=1 or 3 , x3=4or 2 orx1=3 , x2=2 , x3=1Sales is 140.11.3-3.A college student has 7 days remaining before final examinations begin in her four courses, and she wants to allocate this study time as effectively as possible. She needs at least 1 day on each course, and she likes to concentrate on just one course each day, so she wants to allocate 1, 2, 3, or 4 days to each course. Having recently taken an OR course, she decides to use dynamic programming to make these allocations to maximize the total grade points to be obtained from the four courses. She estimates that the alternative allocations for each course would yield the number of grade points shown in the following table:Estimated Grade PointsCourseStudy Days 1 2 3 41 3 52 6 2 5 5 4 73 6 6 7 9 47989Solve this problem by dynamic programming.Solution:Let the s n =the number of day still available for allocation to remaining course(n=1,2,3,4) x n =the number of day allocation to course ns 1=7 1≤x 1≤s 1 s 2=7-x 1 1≤ x 2≤s 2 s 3=7-x 1−x 2 1≤ x 3≤s 3 s 4=7-x 1−x 2−x 3 1≤ x 4=s 4s 2=s 1-x 1 s 3=s 2−x 2 s 4=s 3−x 3P(s i , x i ) is the measure of performance Maximize = ∑P(s i ,x i )4i=1f n ∗s n =max{ P(s n ,x n )+ f n+1∗s n+1} f 5∗s 5=0 s 4f 4x 41 6 12 7 23 9 34 94 fs 3 x 3 1 2 3 4 f 3x 32 8 8 13 9 10 10 24 11 11 13 13 35 11131414143 or 4fs 2 x 2 1 2 3 4 f 2x 23 13 13 1 4151315 15181514181 619181617191s1 x11234f1x1 722232120232Sox1=2,x2=1,x3=3,x4=1The total grade point is 23.11.3-4.A political campaign is entering its final stage, and polls indicate a very close election. One of the candidates has enough funds left to purchase TV time for a total of five prime-time commercials on TV stations located in four different areas. Based on polling information, an estimate has been made of the number of additional votes that can be won in the different broadcasting areas depending upon the number of commercials run. These estimates are given in the following table in thousands of votes:AreaCommercials 1 2 3 40 0 0 0 01 4 6 5 32 7 8 9 73 9 10 11 124 12 11 10 145 15 12 9 16Use dynamic programming to determine how the five commercials should be distributed among the four areas in order to maximize the estimated number of votes won.Solution:Let the s n=the number of commercials still available for allocation to remaining area (n=1,2,3,4) x n=the number of commercials allocation to area ns1=5 0≤x1≤s1s2=5-x1 0≤x2≤s2s3=5-x1−x20≤x3≤s3s 4=5-x 1−x 2−x 3 0≤ x 4=s 4s 2=s 1-x 1 s 3=s 2−x 2 s 4=s 3−x 3P(s i , x i ) is the measure of performance Maximize = ∑P(s i ,x i )4i=1f n ∗s n =max{ P(s n ,x n )+ f n+1∗s n+1} f 5∗s 5=0 s 4f 4x 40 0 0 1 3 1 2 7 2 3 12 3 4 14 4 5 165fs 3 x 3 0 1 2 3 4 5 f 3x 30 0 0 0 1 3 5 5 1 2 7 8 9 9 2 3 12 12 12 11 12 0or1or2 4 14 17 16 14 10 17 1 5 1619211819921 2fs 2 x 2 0 1 2 3 4 5 f 2x 2 0 0 0 0 1 5 6 6 1 2 9 11 8 11 1 3 12 15 13 10 15 1 4 17 18 17 15 11 18 1 5 21 232019161223 1fs 1 x 1 0 1 2 3 4 5 f 1 x 1 5 23222220181523 0So x 1=0,x 2=1,x 3=1,x 4=3, the won number of votes is 23.11.3-5.A county chairwoman of a certain political party is making plans for an upcoming presidential election. She has received the services of six volunteer workers for precinct work, and she wants to assign them to four precincts in such a way as to maximize their effectiveness. She feels that it would be inefficient to assign a worker to more than one precinct, but she is willing to assign no workers to any one of the precincts if they can accomplish more in other precincts.The following table gives the estimated increase in the number of votes for the party's candidate in each precinct if it were allocated various numbers of workers:PrecinctWorkers 1 2 3 40 0 0 0 01 4 7 5 62 9 11 10 113 15 16 15 144 18 18 18 165 22 20 21 176 24 21 22 18This problem has several optimal solutions for how many of the six workers should be assigned to each of the four precincts to maximize the total estimated increase in the plurality of the party's candidate. Use dynamic programming to find all of them so the chairwoman can make the final selection based on other factors.Solution:let the s n=the number of workers still available for allocation to remaining precinct (n=1,2,3,4)x n=the number of workers allocation to precinct ns1=6 0≤x1≤s1s2=6-x1 0≤x2≤s2s3=6-x1−x20≤x3≤s3s4=6-x1−x2−x30≤x4=s4s 2=s 1-x 1 s 3=s 2−x 2 s 4=s 3−x 3P(s i , x i ) is the measure of performance Maximize = ∑P(s i ,x i )4i=1f n ∗s n =max{ P(s n ,x n )+ f n+1∗s n+1} f 5∗s 5=0 s 4f 4x 40 0 0 1 6 1 2 11 2 3 14 3 4 16 4 5 17 5 6 186fs 3 x 3 0 1 2 3 4 5 6 f 3x 30 0 0 0 1 6 5 6 0 2 11 11 10 11 0or1 3 14 16 16 15 16 1or2 4 16 19 21 21 18 21 2or3 5 17 21 24 26 24 21 26 3 6 18 22262929272229 3or4 fs 2 x 2 01 2 3 4 5 6 f 2x 2 0 0 0 0 1 6 7 7 1 2 11 13 11 13 1 3 16 18 17 16 18 1 4 21 23 22 22 18 23 1 5 26 28 27 27 24 20 28 1 6 2933323229262133 1fs 3 x 3 0 1 2 3 4 5 6 f 3 x 3 6 33 32323331292433 0or3So the optimal solutions followx 1=0,x2=1,x3=3,x4=2 orx1=3,x2=1,x3=1,x4=1 orx1=3,x2=1,x3=0,x4=2The total estimated increase in the plurality of the party's candidate is 33.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
CHAPTER 33.1-5、Use the graphical method to solve the problem:Maximize Z=2 x1+ x2Subject toX2<=102 x1+5 x2<=60X1+x2<=183 x1 +x2<=44AndX1>=0,x2>=0Solution:X22218(11.3 , 10)1210Feasible region14.61830X1As the figure above shows, the corner-point is the optimal solution, x1=11.3 and x2=10.3.1-13、consider the following problem, where the value of k has not yet been ascertained.Maximize Z=x1+2 x2,Subject to-x1+x2<=2X2<=3K x1+x2<=2k+3, where k>=0AndX1>=0, x2>=0The solution currently being used is x1=2,x2=3. Use graphical analysis to determine the values of k such that this solution actually is optimal.Solution:X2Corner-point322X1As the picture above shows, the value of k is boundless,any value is ok.3.1-14、consider the following problem, where the value of c1 and c2 has not yet been ascertained.Maximize Z=c1 x1+c2 x2Subject to2x1+ x2<=11-x1+2x2<=2AndX1>=0, x2>=0Use graphical analysis to determine the optimal solution for(x1, x2) for the various possible values of c1 and c2.(hint: separate the cases where c2=0.c2>0,andc2<0.for the latter two case,Focus on the ratio of c1 to c2.)X211(4, 3)15.5X1If the value of c2 is 0, the optimal solution is x1=5, x2=0;If the value of c2 is greater than zero and c1/c2 great than 2, the optimal solution is still x1=5, x2=0;If the value of c2 is greater than zero and c1/c2 equal 2, the optimal solution hasmultiple optimal solutions;If the value of c2 is greater than zero and c1/c2 less than 2, the optimal solution is x1=4, x2=3;If the value of c2 is less than zero and c1/c2 less than 1/2, the optimal solution is x1=4, x2=3;If the value of c2 is less than zero and c1/c2 equal 1/2, the optimal solution has multiple optimal solutions;If the value of c2 is less than zero and c1/c2 greater than 1/2, the optimal solution is x1=0, x2=1;3.4-10. Larry Edison is the director of the Computer Center for Buckly College. He now needs to schedule the staffing of the center. It is open from 8 A.M. until midnight. Larry has monitored the usage of the center at various times of the day, and determined that the following number of computer consultants are required.Time of Day Minimum Number of Consultants Required to Be Duty8 A.M.-noon Noon-4 P.M.4 P.M.-8 P.M.8 P.M.-midnight4 8 10 6Two types of computer consultants can be hired: full-time and part-time. The full-time consultants work for 8 consecutive hours in any of the following shifts: morning (8 A.M.-4 P.M.), afternoon (noon-8 P.M.), and evening (4 P.M.-midnight).Full-time consultants are paid $40 per hour.Part-time consultants can be hired to work any of the four shifts listed in the above table. Part-time consultants are paid $30 per hour.An additional requirement is that during every time period, there must be at least 2 full-time consultants on duty.Larry would like to determine how many full-time and how many part-time workers should work each shift to meet the above requirements at the minimum possible cost.(a)Formulate a liner programming model for this problem.C(b) Solve this model by the simplex method.(不会…希望老师多讲讲)Solution:let the full-time consultants be x1、x2、x3, let the part-time consultants bex4、x5、x6、x7.Min z=40(x1+x2+x3)+30(x4+x5+x6+x7)Subject to:X1>=2X2>=2x3>=2X1+x4>=4X1+x2+x5>=8X2+x3+X6>=10X3+x7>=6AndXj(j=1,2,3,4,5,6,7)>=03.4-11.* The Medequip Company produces precision medical diagnostic equipment at two factories. Three medical centers have placed orders for this month’s production output. The table below shows what the cost would be for shipping each unit from each factory to each of these customers. Also shown are the number of units that will be produced at each factory and the number of units ordered by each customer.ToFromUnit Shipping CostOutputCustomer 1 Customer2 Customer3Factory 1 Factory 2 $600 $800 $700$400 $900 $600400 units500 unitsOrder size 300 units 200 units 400 unitsA decision now needs to be made about the shipping plan for how many units to ship from each factory to each customer.(a)Formulate a liner programing model for this problem.C(b) Solve this model by the simplex method.(同上…)Solution: let factory1 to each customer be x1、x2、x3, let factory2 to each customer be x3、x4、x5.Min z=600x1+800x2+700x3+400x4+900x5+600x6Subject to:X1+x4=300X2+x5=200X3+x6=400X1+x2+x3=400X5+x6+x7=500 AndXj(j=1,2,3,4,5,6)>=0。
