Fast Excitation and Photon Emission of a Single-Atom-Cavity System

合集下载

双光子显微镜的应用优势与维护要素

综述①中山大学中山医学院科研仪器中心广东广州 510080*通信作者：**************作者简介：李娟，女，(1983- )，硕士，助理实验师，从事科研仪器共享服务与管理工作。

中国医学装备2021年12月第18卷第12期 China Medical Equipment 2021 December V ol.18 No.12双光子显微镜是结合了激光扫描共聚焦显微镜及双光子激发技术的一种新精密仪器。

在激光扫描显微镜的基础上，双光子显微成像技术以红外飞秒激光作为光源，受散射影响较小，易穿透样本，可深入组织内部非线性地激发荧光，减小激光对生物体的损伤，光毒性小且具有高空间分辨率，适合生物样品的深层成像及活体样品的长时间观察成像[1-2]。

双光子显微镜已成为生命科学各领域重要的研究工具，可在细胞甚至是亚细胞水平对活体动物的神经细胞形态结构、离子浓度、细胞运动、分子相互作用等生理现象进行直接的长时间成像监测，还能进行光激活染及光损伤等光学操纵，广泛应用于脑科学、免疫学、肿瘤、胚胎发育等生物医学相关研究。

通过阐述双光子显微镜的工作原理、样品前期准备、成像难点及设备使用及日常维护要点，梳理双光子激光共聚焦与其他同类成像类设备在成像原理、配置参数、成像特点及应用领域等方面的不同，为使用者提供更多实验方法参考，使之更好地服务于医学临床、教学和科研。

1 双光子显微镜成像技术原理、优势及应用范围1.1 双光子激发技术的基本原理双光子激发理论由诺贝尔奖得主Goppert Mayer于1931年提出，1961年得到了实验验证[3-4]。

该技术的基本原理是：在高光子密度情况下，荧光分子可同时吸收2个长波长的光子，其效果与使用一个波长为长波长一半的光子去激发荧光分子相同。

长波长的光受散射影响小于短波长的光，易穿透标本；长波长的近红外光对细胞毒性小于短波长的光。

此外，双光子激发要较高的光子密度，为不损伤细胞，双光子显微镜使用高能量锁模脉冲激光器，激光具有高峰值能量及低平均能量，物镜将脉冲激光的光子聚焦时，物镜焦点处的光子密度最高，故双光子激发只发生在物镜的焦点处，只有在焦点平面上才有光漂白及光毒性，所以双光子显微镜无需共聚焦针孔，提高了荧光检测效率。

激光扫描共聚焦显微镜技术

多通道同时检测，可实时检测细胞的生理活动和形态变化:
• 生理学研究：如细胞内各种离子浓度随时间的变化情况.
• 活细胞多种标记物同时进行成像，动态观察不同形态学事件的发生。如分泌颗粒的分泌过程。Leabharlann 三、激光扫描共聚焦显微镜的应用
vestigial apterous CiD (cyanine 5).
透明质酸
• The role of hyaluronan in renal stone disease
• Hyaluronan is expressed by proliferating renal tubular cells in subconfluent cultures (2 days post-seeding). At cell-cell contact (4 days post-seeding) this staining starts to fade away to completely disappear when the tight junctions are assembled (5-6 days post-seeding). The hyaluronan receptor CD44 is also expressed at the luminal surface in subconfluent cultures (2 days post-seeding), at cell-cell contact CD44 is targeted to lateral spaces, whereas at confluence (6 days post-seeding), CD44 is exclusively expressed at basal domains of the plasma membrane.

荧光寿命测试技术-时域或频域

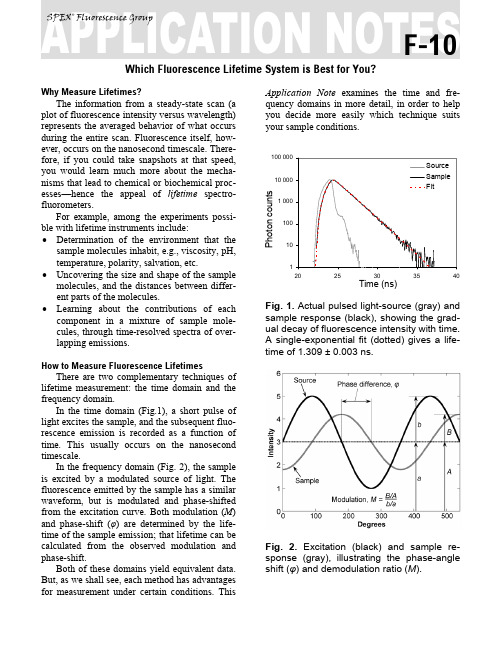
Which Fluorescence Lifetime System is Best for You?Why Measure Lifetimes?The information from a steady-state scan (a plot of fluorescence intensity versus wavelength) represents the averaged behavior of what occurs during the entire scan. Fluorescence itself, how-ever, occurs on the nanosecond timescale. There-fore, if you could take snapshots at that speed, you would learn much more about the mecha-nisms that lead to chemical or biochemical proc-esses—hence the appeal of lifetime spectro-fluorometers.For example, among the experiments possi-ble with lifetime instruments include: •Determination of the environment that the sample molecules inhabit, e.g., viscosity, pH, temperature, polarity, salvation, etc. •Uncovering the size and shape of the sample molecules, and the distances between differ-ent parts of the molecules.•Learning about the contributions of each component in a mixture of sample mole-cules, through time-resolved spectra of over-lapping emissions.How to Measure Fluorescence LifetimesThere are two complementary techniques of lifetime measurement: the time domain and the frequency domain.In the time domain (Fig.1), a short pulse of light excites the sample, and the subsequent fluo-rescence emission is recorded as a function of time. This usually occurs on the nanosecond timescale.In the frequency domain (Fig. 2), the sample is excited by a modulated source of light. The fluorescence emitted by the sample has a similar waveform, but is modulated and phase-shifted from the excitation curve. Both modulation (M) and phase-shift (φ) are determined by the life-time of the sample emission; that lifetime can be calculated from the observed modulation and phase-shift.Both of these domains yield equivalent data. But, as we shall see, each method has advantages for measurement under certain conditions. This Application Note examines the time and fre-quency domains in more detail, in order to help you decide more easily which technique suits your sample conditions.Fig. 1. Actual pulsed light-source (gray) and sample response (black), showing the grad-ual decay of fluorescence intensity with time.A single-exponential fit (dotted) gives a life-time of 1.309 ± 0.003 ns.Fig. 2. Excitation (black) and sample re-sponse (gray), illustrating the phase-angle shift (φ) and demodulation ratio (M).SPEX® Fluorescence GroupF-10Fluorescence Decay LawBoth the time- and frequency-domain meth-ods take advantage of the fluorescence decay law , which is based on first-order kinetics. The decay law postulates that if a population of mole-cules is instantaneously excited when photons are absorbed, then the excited population—and hence the fluorescence intensity as a function of time, I (t )—gradually decays to the ground state. Decay kinetics can be described byταte t I −=)(where α is the intensity at time t = 0, t is the time after the absorption, and τ is the lifetime, that is, when the fraction of the population of molecules in the excited state (and the fluorescence inten-sity) has decreased by a factor of 1/e, or ~37%. Note that before absorption, I (t ) = 0.This fluorescence decay law implies that all excited molecules exist in a homogenous envi-ronment, as is true for many single-exponential fluorescence lifetime standards in solution 1,2. Apart from such standards, however, single-ex-ponential decays are usually a real-life exception, because most populations of excited molecules do not exist in homogeneous environments, and can be influenced by various factors, including the presence of quenchers , energy-transfer proc-esses among members of the population, and dif-ferent rates of molecular rotation. Hence, in most instances, multi-exponential or non-exponential forms of the decay-law equation must be applied.Time DomainTime-domain measurements are based on the assumption that, when photons are absorbed, the molecules can be excited in an infinitely brief moment. This idea is commonly known as the delta or δ-pulse . The δ-pulse idea is used to in-terpret data obtained with real pulsed light-sources with measurable pulse-widths. In prac-tice, the time-dependent profile of the light-pulse is reconvolved with the decay-law function. Re-convolution assumes that the δ-pulses are con-tinuous functions, so that the observed decay is the convolution integral of the decays from all δ-pulses initiated during the finite pulse-width 3.Fig. 3. Time-Correlated Single-Photon-Counting fluorometer. A pulsed light Source excites the Sample repetitively. The sample emission is observed by a Detector, while the excitation flashes are detected by a syn-chronization module (SYNC). A constant-fraction discriminator (CFD) responds to only the first photon detected (small ar-rows)—independent of its amplitude—from the detector. This first photon from sample emission is the stop signal for the Time-to-Amplitude Converter (TAC). The excitation pulses trigger the start signals. The Multi-Channel Analyzer (MCA) records repetitive start-stop signals of the single-photon events from the TAC, to generate a histo-gram of photon counts as a function of time-channel units. The lifetime is calculated from this histogram.There are many ways to record time-domain data, such as streak cameras, boxcar integrators,19202122232425262728TIME, CHANNELSCumulative histogramMonochroma-Sample chamberand so forth. Most agree, however, that the method of single-photon counting is, by far, su-perior.T ime-C orrelated S ingle-P hoton C ounting (TCSPC) measurements are shown in Fig. 3. TCSPC uses a pulsed light-source and a circuit to detect single-photon events at a detector. In a repetitive series of many start-stop signals from the circuitry, a binned histogram in time chan-nels of single-photon counts is gradually gener-ated.TCSPC relies on a principle of Poissonian statistics, that only one photon can be counted at a time and in any one channel, to avoid skewing the time-dependent statistics in photon-pile-up. Pile-up thus limits the data-acquisition rate of TCSPC to a few (typically 1–2) percent of the repetition rate. In practice, the single-photon limit is not a major hindrance because the pile-up limit can be monitored during the experiment, and decay times with sufficient photon counts in can be obtained in seconds to minutes with repe-tition rates in the MHz range. In addition, the Poissonian nature of the statistics allows the data to be rigorously analyzed.Frequency DomainThe fluorescence decay parameters in the de-cay law’s impulse function may be obtained based on the relation of a sinusoidally modulated excitation beam to the fluorescence emission re-sponse (Fig. 2). The emission occurs at the same frequency as the excitation. Because of the loss of electron energy (Stokes’ shift) between exci-tation and emission, the emission waveform is demodulated and phase-shifted in comparison to the excitation. Thus the demodulation ratio (M) and phase-angle shift (φ) constitute two separate observable parameters that are both directly re-lated, via a Fourier transformation4, to the initial fluorescence intensity, α, and lifetime, τ, for a population of fluorophores.Frequency-domain measurements are best performed using M ulti-F requency C ross-C orre-lation phase-and-modulation (MFCC), shown in Fig. 4. A modulated beam excites the sample. The fluorescence emission is detected by a P ho-to M ultiplier T ube (PMT) modulated at the same base radio-frequency as the master plus a low cross-correlation frequency (a few Hz). The base-frequency signals are filtered to reveal the cross-correlation frequency signal, which con-tains all the same demodulation (M) and phase-angle shift (φ) information as the fluorescence emission.Fig. 4. Multi-Frequency Cross-Correlation fluorometer. An unmodulated light Source emits a spectrum of continuous-wave light. The excitation monochromator (Excit. Mono.) selects an excitation wavelength. An amplified (Amp 1) master synthesizer (Mas-ter) drives the Pockels cell (Pockels) at a base frequency, Rf, which modulates the excitation beam. The modulated beam ex-cites the Sample, causing the sample to emit modulated fluorescence also at the base Rf. An emission monochromator (Emis. Mono.) selects one wavelength of modulated fluorescence. The photomultiplier tube (PMT) is modulated by an amplified (Amp 2) slave synthesizer (Slave) at the base Rf plus a low-frequency cross-correla-tion note (∆f). The sample emission at Rf cancels the slave Rf+∆f frequencies to yield the ∆f signal containing the same phase-an-gle shift (φ) and demodulation ratio (M) as the Rf fluorescence.SampleExcit.AMPLITUDE∆fUsually the sample is scanned over a range (10–16) of frequencies because of the intrinsic limitations of resolving mixtures using only one frequency. In theory, for a single-exponential emitter, one should observe the same lifetime using the observed φ and M at any given fre-quency4,5. However, for mixtures of components, φ is biased towards the faster decay components and M is weighted toward the slower compo-nents. Hence, resolving separate fluorescence lifetime parameters for mixtures requires a range of frequencies to be statistically valid. On the other hand, the MFCC technique is not con-strained by the Poissonian single-photon detec-tion limits of TCSPC. Therefore, rapid acquisi-tion at a high signal-to-noise ratio to resolve complex mixtures is possible.Which Method is Best for Your Sample?In principle, both methods can yield identical results for a wide variety of experimental sam-ples and conditions. A user may choose one Spex®-IBH fluorescence lifetime instrument method over the other for a variety of reasons: The frequency domain, for example, is compatible with a strong, tunable continuous-wave xenon excitation source that seamlessly covers a wide spectral range. With the frequency domain, lifetimes as short as 10 picoseconds can be measured with a continuous source, tunable from the UV to the near-IR. All other conditions being equal, frequency domain is usually faster than time domain.Time-domain instruments have no conti-nuously tunable, pulsed source from the UV to IR, so the choice of excitation wavelength is more limited. Because of pulse-width, lifetimes are usually limited to no shorter than just under a nanosecond with a flash lamp, though they can operate at picosecond timeframes with inexpen-sive diode sources. On the other hand, TCSPC is compatible with precise and inexpensive pulsed light-emitting diodes and diode-laser sources. The single-photon detection method is preferred when photon emission is too weak for fre-quency-domain detection. There is a sacrifice in available wavelengths for UV excitation, impor-tant for some biological samples, when the flash lamp is used, and may be inconvenient and slow.Most important for you, Jobin Yvon, uniting Spex® and IBH, now offers both time- and fre-quency-domain instruments to optimize your re-search time and effort. The choice is yours. Copyright © 2004 Jobin Yvon, Inc.1 R.A. Lampert, et al., Anal. Chem.,55:68–73, 1983.2 J.R. Lakowicz, et al., J. Fluor., 1(2):87–93, 1991.3 G. Hungerford and D.J.S. Birch, Meas. Sci. Tech., 7:121–135, 1996.4 D.M. Jameson and T.L. Hazlett, Biophysical and Bio-chemical Aspects of Fluorescence Spectroscopy, Plenum Press, New York, pp. 105–133, 1991.5 E. Gratton, et al., Ann. Rev. Biophys. Bioeng., 13:105–124, 1984.In the USA:Jobin Yvon Inc.3880 Park Avenue, Edison, NJ 08820 In France: Japan: +81 (0) 3 58230140 Tel:+1-732-494-8660 16-18, rue du Canal China: +86 (0) 10 6849 2216 Fax: +1-732-549-5157 91165 Longjumeau cedex Germany: +49 (0) 89 462317-0 E-Mail: info@ Tel: +33 (0) 1 64 54 13 00 Italy: +39 0 2 576047621-800-533-5946Fax: +33 (0) 1 69 09 93 19 U.K.: +44 (0) 8204 8142。

超快光学超快光谱

Chopper
Chopped excite pulse train
The excite pulse periodically changes the sample absorption seen by the probe pulse.
Probe pulse train
Lock-in detector
What’s going on in spectroscopy measurements
The excite pulse(s) excite(s) molecules into excited states, which changes the medium’s absorption coefficient and refractive index.
DT(t) / T0 Da0 exp(–t /tex) L
0
Delay, t
Modeling excite-probe measurements
(cont’d)
3
Excite transition
2 Probe transition
1
0
More complex decays occur if intermediate states are populated or if the motion is complex. Imagine probing an intermediate transition, whose states temporarily fill with molecules on their way back down to the ground state:
Ultrafast laser spectroscopy: Why
Most events that occur in atoms and molecules occur on fs and ps time scales because the length scales are very small.

fluorescence

fluorescenceFluorescence: An Insight into the Phenomenon, Applications, and Future PerspectivesIntroduction:Fluorescence is a fascinating natural phenomenon observed in various living organisms, minerals, and chemicals. It involves the emission of light by a substance after being exposed to electromagnetic radiation, typically ultraviolet (UV) light. This phenomenon has gained immense significance in scientific research and various technological applications. In this document, we will delve into the world of fluorescence, exploring its underlying principles, applications in diverse fields, and future perspectives.1. Principles of Fluorescence:Fluorescence revolves around the absorption and subsequent re-emission of light within the visible spectrum. When a fluorophore, a substance capable of fluorescing, absorbs energy from a photon, electrons within the molecule get excited and move to higher energy levels. As the electronsreturn to their ground state, they emit light of lower energy, resulting in fluorescence. This emission occurs at longer wavelengths than the absorbed light, thus producing a characteristic color glow.2. Exploring Fluorescent Probes:Fluorescent probes are crucial tools used to visualize and track specific molecules or cellular structures in biological systems. These probes are designed to selectively bind to the target of interest and emit fluorescence upon excitation. From traditional organic dyes to advanced quantum dots and genetically encoded fluorescent proteins, a wide range of probes have been developed to cater to various research needs. These probes have revolutionized biological imaging, enabling scientists to study cellular processes with high specificity and sensitivity.3. Applications in Biology and Medicine:Fluorescence imaging techniques, such as fluorescence microscopy and flow cytometry, have become cornerstones of modern biological research. They allow direct visualization and quantification of cellular events, such as protein localization, gene expression, and cellular interactions.Additionally, fluorescence-based methods are vital in clinical diagnostics, such as immunoassays, DNA sequencing, and fluorescence in situ hybridization (FISH). These techniques have facilitated early disease detection, drug discovery, and personalized medicine, leading to significant advancements in healthcare.4. Industrial and Environmental Applications:Fluorescence finds applications beyond the realm of biology and medicine. Industries harness its potential in various technologies and processes. For instance, fluorescence is exploited in the analysis of food and beverage quality, environmental monitoring, and detection of contaminants. The use of fluorescence in chemical sensors and biosensors has facilitated rapid and sensitive detection of analytes, helping ensure product safety and environmental health.5. Future Perspectives:As technology continues to advance, the field of fluorescence is poised for exciting developments. Researchers are actively exploring novel fluorophores with enhanced properties, such as brighter emission, improved photostability, and longer fluorescence lifetimes. The integration of fluorescencetechniques with other cutting-edge technologies, like super-resolution microscopy and optogenetics, holds great potential for pushing the boundaries of scientific discovery. Furthermore, the emerging field of nanotechnology promises innovative applications of fluorescence, including targeted drug delivery systems and ultra-sensitive biosensors.Conclusion:Fluorescence is a captivating phenomenon that has revolutionized scientific research and technological advancements. From its fundamental principles to its diverse applications, fluorescence has transformed the way we understand and interact with the world around us. Its contribution to biology, medicine, industry, and the environment cannot be overstated. As we move forward, embracing the rapid advancements in the field, the future of fluorescence holds immense promise for furthering human knowledge and improving our lives in countless ways.。

7. Emission and Absorption

abs dPki = Bki wν (ν) (7.2) dt is proportional to the spectral energy density wν (ν) = n (ν)h ν of the radiation ﬁeld (where n (ν) is the number of photons h ν per unit volume within the frequency interval ∆ν = 1 s−1 ). The proportionality factor Bki is the Einstein coefﬁcient for absorption. Each absorption takes one photon from a speciﬁc mode of the radiation ﬁeld (see Sect. 3.1) and therefore decreases the number of photons in this mode by one. The radiation ﬁeld can also induce atoms in an excited state with energy E i to emit a photon with energy h ν = E i − E k into a speciﬁc mode of the radiation ﬁeld and to go into the lower state E k . This process is called induced (or stimulated) emission. It increases by one the number of photons in this mode from which the inducing photon came. Since the two photons are in the same mode, they have identical propagation directions. The energy of the atom is reduced by ∆ E and that of

物理学专业英语

华中师范大学物理学院物理学专业英语仅供内部学习参考！2014一、课程的任务和教学目的通过学习《物理学专业英语》，学生将掌握物理学领域使用频率较高的专业词汇和表达方法，进而具备基本的阅读理解物理学专业文献的能力。

通过分析《物理学专业英语》课程教材中的范文，学生还将从英语角度理解物理学中个学科的研究内容和主要思想，提高学生的专业英语能力和了解物理学研究前沿的能力。

培养专业英语阅读能力，了解科技英语的特点，提高专业外语的阅读质量和阅读速度；掌握一定量的本专业英文词汇，基本达到能够独立完成一般性本专业外文资料的阅读；达到一定的笔译水平。

要求译文通顺、准确和专业化。

二、课程内容课程内容包括以下章节：物理学、经典力学、热力学、电磁学、光学、原子物理、统计力学、量子力学和狭义相对论三、基本要求1.充分利用课内时间保证充足的阅读量（约1200~1500词/学时），要求正确理解原文。

2.泛读适量课外相关英文读物，要求基本理解原文主要内容。

3.掌握基本专业词汇（不少于200词）。

4.应具有流利阅读、翻译及赏析专业英语文献，并能简单地进行写作的能力。

四、参考书目录1 Physics 物理学 (1)Introduction to physics (1)Classical and modern physics (2)Research fields (4)V ocabulary (7)2 Classical mechanics 经典力学 (10)Introduction (10)Description of classical mechanics (10)Momentum and collisions (14)Angular momentum (15)V ocabulary (16)3 Thermodynamics 热力学 (18)Introduction (18)Laws of thermodynamics (21)System models (22)Thermodynamic processes (27)Scope of thermodynamics (29)V ocabulary (30)4 Electromagnetism 电磁学 (33)Introduction (33)Electrostatics (33)Magnetostatics (35)Electromagnetic induction (40)V ocabulary (43)5 Optics 光学 (45)Introduction (45)Geometrical optics (45)Physical optics (47)Polarization (50)V ocabulary (51)6 Atomic physics 原子物理 (52)Introduction (52)Electronic configuration (52)Excitation and ionization (56)V ocabulary (59)7 Statistical mechanics 统计力学 (60)Overview (60)Fundamentals (60)Statistical ensembles (63)V ocabulary (65)8 Quantum mechanics 量子力学 (67)Introduction (67)Mathematical formulations (68)Quantization (71)Wave-particle duality (72)Quantum entanglement (75)V ocabulary (77)9 Special relativity 狭义相对论 (79)Introduction (79)Relativity of simultaneity (80)Lorentz transformations (80)Time dilation and length contraction (81)Mass-energy equivalence (82)Relativistic energy-momentum relation (86)V ocabulary (89)正文标记说明：蓝色Arial字体（例如energy）：已知的专业词汇蓝色Arial字体加下划线（例如electromagnetism）：新学的专业词汇黑色Times New Roman字体加下划线（例如postulate）：新学的普通词汇1 Physics 物理学1 Physics 物理学Introduction to physicsPhysics is a part of natural philosophy and a natural science that involves the study of matter and its motion through space and time, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic disciplines, perhaps the oldest through its inclusion of astronomy. Over the last two millennia, physics was a part of natural philosophy along with chemistry, certain branches of mathematics, and biology, but during the Scientific Revolution in the 17th century, the natural sciences emerged as unique research programs in their own right. Physics intersects with many interdisciplinary areas of research, such as biophysics and quantum chemistry,and the boundaries of physics are not rigidly defined. New ideas in physics often explain the fundamental mechanisms of other sciences, while opening new avenues of research in areas such as mathematics and philosophy.Physics also makes significant contributions through advances in new technologies that arise from theoretical breakthroughs. For example, advances in the understanding of electromagnetism or nuclear physics led directly to the development of new products which have dramatically transformed modern-day society, such as television, computers, domestic appliances, and nuclear weapons; advances in thermodynamics led to the development of industrialization; and advances in mechanics inspired the development of calculus.Core theoriesThough physics deals with a wide variety of systems, certain theories are used by all physicists. Each of these theories were experimentally tested numerous times and found correct as an approximation of nature (within a certain domain of validity).For instance, the theory of classical mechanics accurately describes the motion of objects, provided they are much larger than atoms and moving at much less than the speed of light. These theories continue to be areas of active research, and a remarkable aspect of classical mechanics known as chaos was discovered in the 20th century, three centuries after the original formulation of classical mechanics by Isaac Newton (1642–1727) 【艾萨克·牛顿】.University PhysicsThese central theories are important tools for research into more specialized topics, and any physicist, regardless of his or her specialization, is expected to be literate in them. These include classical mechanics, quantum mechanics, thermodynamics and statistical mechanics, electromagnetism, and special relativity.Classical and modern physicsClassical mechanicsClassical physics includes the traditional branches and topics that were recognized and well-developed before the beginning of the 20th century—classical mechanics, acoustics, optics, thermodynamics, and electromagnetism.Classical mechanics is concerned with bodies acted on by forces and bodies in motion and may be divided into statics (study of the forces on a body or bodies at rest), kinematics (study of motion without regard to its causes), and dynamics (study of motion and the forces that affect it); mechanics may also be divided into solid mechanics and fluid mechanics (known together as continuum mechanics), the latter including such branches as hydrostatics, hydrodynamics, aerodynamics, and pneumatics.Acoustics is the study of how sound is produced, controlled, transmitted and received. Important modern branches of acoustics include ultrasonics, the study of sound waves of very high frequency beyond the range of human hearing; bioacoustics the physics of animal calls and hearing, and electroacoustics, the manipulation of audible sound waves using electronics.Optics, the study of light, is concerned not only with visible light but also with infrared and ultraviolet radiation, which exhibit all of the phenomena of visible light except visibility, e.g., reflection, refraction, interference, diffraction, dispersion, and polarization of light.Heat is a form of energy, the internal energy possessed by the particles of which a substance is composed; thermodynamics deals with the relationships between heat and other forms of energy.Electricity and magnetism have been studied as a single branch of physics since the intimate connection between them was discovered in the early 19th century; an electric current gives rise to a magnetic field and a changing magnetic field induces an electric current. Electrostatics deals with electric charges at rest, electrodynamics with moving charges, and magnetostatics with magnetic poles at rest.Modern PhysicsClassical physics is generally concerned with matter and energy on the normal scale of1 Physics 物理学observation, while much of modern physics is concerned with the behavior of matter and energy under extreme conditions or on the very large or very small scale.For example, atomic and nuclear physics studies matter on the smallest scale at which chemical elements can be identified.The physics of elementary particles is on an even smaller scale, as it is concerned with the most basic units of matter; this branch of physics is also known as high-energy physics because of the extremely high energies necessary to produce many types of particles in large particle accelerators. On this scale, ordinary, commonsense notions of space, time, matter, and energy are no longer valid.The two chief theories of modern physics present a different picture of the concepts of space, time, and matter from that presented by classical physics.Quantum theory is concerned with the discrete, rather than continuous, nature of many phenomena at the atomic and subatomic level, and with the complementary aspects of particles and waves in the description of such phenomena.The theory of relativity is concerned with the description of phenomena that take place in a frame of reference that is in motion with respect to an observer; the special theory of relativity is concerned with relative uniform motion in a straight line and the general theory of relativity with accelerated motion and its connection with gravitation.Both quantum theory and the theory of relativity find applications in all areas of modern physics.Difference between classical and modern physicsWhile physics aims to discover universal laws, its theories lie in explicit domains of applicability. Loosely speaking, the laws of classical physics accurately describe systems whose important length scales are greater than the atomic scale and whose motions are much slower than the speed of light. Outside of this domain, observations do not match their predictions.Albert Einstein【阿尔伯特·爱因斯坦】contributed the framework of special relativity, which replaced notions of absolute time and space with space-time and allowed an accurate description of systems whose components have speeds approaching the speed of light.Max Planck【普朗克】, Erwin Schrödinger【薛定谔】, and others introduced quantum mechanics, a probabilistic notion of particles and interactions that allowed an accurate description of atomic and subatomic scales.Later, quantum field theory unified quantum mechanics and special relativity.General relativity allowed for a dynamical, curved space-time, with which highly massiveUniversity Physicssystems and the large-scale structure of the universe can be well-described. General relativity has not yet been unified with the other fundamental descriptions; several candidate theories of quantum gravity are being developed.Research fieldsContemporary research in physics can be broadly divided into condensed matter physics; atomic, molecular, and optical physics; particle physics; astrophysics; geophysics and biophysics. Some physics departments also support research in Physics education.Since the 20th century, the individual fields of physics have become increasingly specialized, and today most physicists work in a single field for their entire careers. "Universalists" such as Albert Einstein (1879–1955) and Lev Landau (1908–1968)【列夫·朗道】, who worked in multiple fields of physics, are now very rare.Condensed matter physicsCondensed matter physics is the field of physics that deals with the macroscopic physical properties of matter. In particular, it is concerned with the "condensed" phases that appear whenever the number of particles in a system is extremely large and the interactions between them are strong.The most familiar examples of condensed phases are solids and liquids, which arise from the bonding by way of the electromagnetic force between atoms. More exotic condensed phases include the super-fluid and the Bose–Einstein condensate found in certain atomic systems at very low temperature, the superconducting phase exhibited by conduction electrons in certain materials,and the ferromagnetic and antiferromagnetic phases of spins on atomic lattices.Condensed matter physics is by far the largest field of contemporary physics.Historically, condensed matter physics grew out of solid-state physics, which is now considered one of its main subfields. The term condensed matter physics was apparently coined by Philip Anderson when he renamed his research group—previously solid-state theory—in 1967. In 1978, the Division of Solid State Physics of the American Physical Society was renamed as the Division of Condensed Matter Physics.Condensed matter physics has a large overlap with chemistry, materials science, nanotechnology and engineering.Atomic, molecular and optical physicsAtomic, molecular, and optical physics (AMO) is the study of matter–matter and light–matter interactions on the scale of single atoms and molecules.1 Physics 物理学The three areas are grouped together because of their interrelationships, the similarity of methods used, and the commonality of the energy scales that are relevant. All three areas include both classical, semi-classical and quantum treatments; they can treat their subject from a microscopic view (in contrast to a macroscopic view).Atomic physics studies the electron shells of atoms. Current research focuses on activities in quantum control, cooling and trapping of atoms and ions, low-temperature collision dynamics and the effects of electron correlation on structure and dynamics. Atomic physics is influenced by the nucleus (see, e.g., hyperfine splitting), but intra-nuclear phenomena such as fission and fusion are considered part of high-energy physics.Molecular physics focuses on multi-atomic structures and their internal and external interactions with matter and light.Optical physics is distinct from optics in that it tends to focus not on the control of classical light fields by macroscopic objects, but on the fundamental properties of optical fields and their interactions with matter in the microscopic realm.High-energy physics (particle physics) and nuclear physicsParticle physics is the study of the elementary constituents of matter and energy, and the interactions between them.In addition, particle physicists design and develop the high energy accelerators,detectors, and computer programs necessary for this research. The field is also called "high-energy physics" because many elementary particles do not occur naturally, but are created only during high-energy collisions of other particles.Currently, the interactions of elementary particles and fields are described by the Standard Model.●The model accounts for the 12 known particles of matter (quarks and leptons) thatinteract via the strong, weak, and electromagnetic fundamental forces.●Dynamics are described in terms of matter particles exchanging gauge bosons (gluons,W and Z bosons, and photons, respectively).●The Standard Model also predicts a particle known as the Higgs boson. In July 2012CERN, the European laboratory for particle physics, announced the detection of a particle consistent with the Higgs boson.Nuclear Physics is the field of physics that studies the constituents and interactions of atomic nuclei. The most commonly known applications of nuclear physics are nuclear power generation and nuclear weapons technology, but the research has provided application in many fields, including those in nuclear medicine and magnetic resonance imaging, ion implantation in materials engineering, and radiocarbon dating in geology and archaeology.University PhysicsAstrophysics and Physical CosmologyAstrophysics and astronomy are the application of the theories and methods of physics to the study of stellar structure, stellar evolution, the origin of the solar system, and related problems of cosmology. Because astrophysics is a broad subject, astrophysicists typically apply many disciplines of physics, including mechanics, electromagnetism, statistical mechanics, thermodynamics, quantum mechanics, relativity, nuclear and particle physics, and atomic and molecular physics.The discovery by Karl Jansky in 1931 that radio signals were emitted by celestial bodies initiated the science of radio astronomy. Most recently, the frontiers of astronomy have been expanded by space exploration. Perturbations and interference from the earth's atmosphere make space-based observations necessary for infrared, ultraviolet, gamma-ray, and X-ray astronomy.Physical cosmology is the study of the formation and evolution of the universe on its largest scales. Albert Einstein's theory of relativity plays a central role in all modern cosmological theories. In the early 20th century, Hubble's discovery that the universe was expanding, as shown by the Hubble diagram, prompted rival explanations known as the steady state universe and the Big Bang.The Big Bang was confirmed by the success of Big Bang nucleo-synthesis and the discovery of the cosmic microwave background in 1964. The Big Bang model rests on two theoretical pillars: Albert Einstein's general relativity and the cosmological principle (On a sufficiently large scale, the properties of the Universe are the same for all observers). Cosmologists have recently established the ΛCDM model (the standard model of Big Bang cosmology) of the evolution of the universe, which includes cosmic inflation, dark energy and dark matter.Current research frontiersIn condensed matter physics, an important unsolved theoretical problem is that of high-temperature superconductivity. Many condensed matter experiments are aiming to fabricate workable spintronics and quantum computers.In particle physics, the first pieces of experimental evidence for physics beyond the Standard Model have begun to appear. Foremost among these are indications that neutrinos have non-zero mass. These experimental results appear to have solved the long-standing solar neutrino problem, and the physics of massive neutrinos remains an area of active theoretical and experimental research. Particle accelerators have begun probing energy scales in the TeV range, in which experimentalists are hoping to find evidence for the super-symmetric particles, after discovery of the Higgs boson.Theoretical attempts to unify quantum mechanics and general relativity into a single theory1 Physics 物理学of quantum gravity, a program ongoing for over half a century, have not yet been decisively resolved. The current leading candidates are M-theory, superstring theory and loop quantum gravity.Many astronomical and cosmological phenomena have yet to be satisfactorily explained, including the existence of ultra-high energy cosmic rays, the baryon asymmetry, the acceleration of the universe and the anomalous rotation rates of galaxies.Although much progress has been made in high-energy, quantum, and astronomical physics, many everyday phenomena involving complexity, chaos, or turbulence are still poorly understood. Complex problems that seem like they could be solved by a clever application of dynamics and mechanics remain unsolved; examples include the formation of sand-piles, nodes in trickling water, the shape of water droplets, mechanisms of surface tension catastrophes, and self-sorting in shaken heterogeneous collections.These complex phenomena have received growing attention since the 1970s for several reasons, including the availability of modern mathematical methods and computers, which enabled complex systems to be modeled in new ways. Complex physics has become part of increasingly interdisciplinary research, as exemplified by the study of turbulence in aerodynamics and the observation of pattern formation in biological systems.Vocabulary★natural science 自然科学academic disciplines 学科astronomy 天文学in their own right 凭他们本身的实力intersects相交，交叉interdisciplinary交叉学科的，跨学科的★quantum 量子的theoretical breakthroughs 理论突破★electromagnetism 电磁学dramatically显著地★thermodynamics热力学★calculus微积分validity★classical mechanics 经典力学chaos 混沌literate 学者★quantum mechanics量子力学★thermodynamics and statistical mechanics热力学与统计物理★special relativity狭义相对论is concerned with 关注，讨论，考虑acoustics 声学★optics 光学statics静力学at rest 静息kinematics运动学★dynamics动力学ultrasonics超声学manipulation 操作，处理，使用University Physicsinfrared红外ultraviolet紫外radiation辐射reflection 反射refraction 折射★interference 干涉★diffraction 衍射dispersion散射★polarization 极化，偏振internal energy 内能Electricity电性Magnetism 磁性intimate 亲密的induces 诱导，感应scale尺度★elementary particles基本粒子★high-energy physics 高能物理particle accelerators 粒子加速器valid 有效的，正当的★discrete离散的continuous 连续的complementary 互补的★frame of reference 参照系★the special theory of relativity 狭义相对论★general theory of relativity 广义相对论gravitation 重力，万有引力explicit 详细的，清楚的★quantum field theory 量子场论★condensed matter physics凝聚态物理astrophysics天体物理geophysics地球物理Universalist博学多才者★Macroscopic宏观Exotic奇异的★Superconducting 超导Ferromagnetic铁磁质Antiferromagnetic 反铁磁质★Spin自旋Lattice 晶格，点阵，网格★Society社会，学会★microscopic微观的hyperfine splitting超精细分裂fission分裂，裂变fusion熔合，聚变constituents成分，组分accelerators加速器detectors 检测器★quarks夸克lepton 轻子gauge bosons规范玻色子gluons胶子★Higgs boson希格斯玻色子CERN欧洲核子研究中心★Magnetic Resonance Imaging磁共振成像，核磁共振ion implantation 离子注入radiocarbon dating放射性碳年代测定法geology地质学archaeology考古学stellar 恒星cosmology宇宙论celestial bodies 天体Hubble diagram 哈勃图Rival竞争的★Big Bang大爆炸nucleo-synthesis核聚合，核合成pillar支柱cosmological principle宇宙学原理ΛCDM modelΛ-冷暗物质模型cosmic inflation宇宙膨胀1 Physics 物理学fabricate制造，建造spintronics自旋电子元件，自旋电子学★neutrinos 中微子superstring 超弦baryon重子turbulence湍流，扰动，骚动catastrophes突变，灾变，灾难heterogeneous collections异质性集合pattern formation模式形成University Physics2 Classical mechanics 经典力学IntroductionIn physics, classical mechanics is one of the two major sub-fields of mechanics, which is concerned with the set of physical laws describing the motion of bodies under the action of a system of forces. The study of the motion of bodies is an ancient one, making classical mechanics one of the oldest and largest subjects in science, engineering and technology.Classical mechanics describes the motion of macroscopic objects, from projectiles to parts of machinery, as well as astronomical objects, such as spacecraft, planets, stars, and galaxies. Besides this, many specializations within the subject deal with gases, liquids, solids, and other specific sub-topics.Classical mechanics provides extremely accurate results as long as the domain of study is restricted to large objects and the speeds involved do not approach the speed of light. When the objects being dealt with become sufficiently small, it becomes necessary to introduce the other major sub-field of mechanics, quantum mechanics, which reconciles the macroscopic laws of physics with the atomic nature of matter and handles the wave–particle duality of atoms and molecules. In the case of high velocity objects approaching the speed of light, classical mechanics is enhanced by special relativity. General relativity unifies special relativity with Newton's law of universal gravitation, allowing physicists to handle gravitation at a deeper level.The initial stage in the development of classical mechanics is often referred to as Newtonian mechanics, and is associated with the physical concepts employed by and the mathematical methods invented by Newton himself, in parallel with Leibniz【莱布尼兹】, and others.Later, more abstract and general methods were developed, leading to reformulations of classical mechanics known as Lagrangian mechanics and Hamiltonian mechanics. These advances were largely made in the 18th and 19th centuries, and they extend substantially beyond Newton's work, particularly through their use of analytical mechanics. Ultimately, the mathematics developed for these were central to the creation of quantum mechanics.Description of classical mechanicsThe following introduces the basic concepts of classical mechanics. For simplicity, it often2 Classical mechanics 经典力学models real-world objects as point particles, objects with negligible size. The motion of a point particle is characterized by a small number of parameters: its position, mass, and the forces applied to it.In reality, the kind of objects that classical mechanics can describe always have a non-zero size. (The physics of very small particles, such as the electron, is more accurately described by quantum mechanics). Objects with non-zero size have more complicated behavior than hypothetical point particles, because of the additional degrees of freedom—for example, a baseball can spin while it is moving. However, the results for point particles can be used to study such objects by treating them as composite objects, made up of a large number of interacting point particles. The center of mass of a composite object behaves like a point particle.Classical mechanics uses common-sense notions of how matter and forces exist and interact. It assumes that matter and energy have definite, knowable attributes such as where an object is in space and its speed. It also assumes that objects may be directly influenced only by their immediate surroundings, known as the principle of locality.In quantum mechanics objects may have unknowable position or velocity, or instantaneously interact with other objects at a distance.Position and its derivativesThe position of a point particle is defined with respect to an arbitrary fixed reference point, O, in space, usually accompanied by a coordinate system, with the reference point located at the origin of the coordinate system. It is defined as the vector r from O to the particle.In general, the point particle need not be stationary relative to O, so r is a function of t, the time elapsed since an arbitrary initial time.In pre-Einstein relativity (known as Galilean relativity), time is considered an absolute, i.e., the time interval between any given pair of events is the same for all observers. In addition to relying on absolute time, classical mechanics assumes Euclidean geometry for the structure of space.Velocity and speedThe velocity, or the rate of change of position with time, is defined as the derivative of the position with respect to time. In classical mechanics, velocities are directly additive and subtractive as vector quantities; they must be dealt with using vector analysis.When both objects are moving in the same direction, the difference can be given in terms of speed only by ignoring direction.University PhysicsAccelerationThe acceleration , or rate of change of velocity, is the derivative of the velocity with respect to time (the second derivative of the position with respect to time).Acceleration can arise from a change with time of the magnitude of the velocity or of the direction of the velocity or both . If only the magnitude v of the velocity decreases, this is sometimes referred to as deceleration , but generally any change in the velocity with time, including deceleration, is simply referred to as acceleration.Inertial frames of referenceWhile the position and velocity and acceleration of a particle can be referred to any observer in any state of motion, classical mechanics assumes the existence of a special family of reference frames in terms of which the mechanical laws of nature take a comparatively simple form. These special reference frames are called inertial frames .An inertial frame is such that when an object without any force interactions (an idealized situation) is viewed from it, it appears either to be at rest or in a state of uniform motion in a straight line. This is the fundamental definition of an inertial frame. They are characterized by the requirement that all forces entering the observer's physical laws originate in identifiable sources (charges, gravitational bodies, and so forth).A non-inertial reference frame is one accelerating with respect to an inertial one, and in such a non-inertial frame a particle is subject to acceleration by fictitious forces that enter the equations of motion solely as a result of its accelerated motion, and do not originate in identifiable sources. These fictitious forces are in addition to the real forces recognized in an inertial frame.A key concept of inertial frames is the method for identifying them. For practical purposes, reference frames that are un-accelerated with respect to the distant stars are regarded as good approximations to inertial frames.Forces; Newton's second lawNewton was the first to mathematically express the relationship between force and momentum . Some physicists interpret Newton's second law of motion as a definition of force and mass, while others consider it a fundamental postulate, a law of nature. Either interpretation has the same mathematical consequences, historically known as "Newton's Second Law":a m t v m t p F ===d )(d d dThe quantity m v is called the (canonical ) momentum . The net force on a particle is thus equal to rate of change of momentum of the particle with time.So long as the force acting on a particle is known, Newton's second law is sufficient to。

一束光的唯美英文

一束光的唯美英文One Beam of LightA beam of light can be an extraordinary sight. The way it illuminates a dark room, casting shadows and creating highlights, can be truly beautiful. But have you ever stopped to consider the wonder of a beam of light? Here, we’ll explore the step-by-step process of how light travels, and why it’s so mesmerizing.Step 1: The Emission of LightThe first step in this journey is the emission of light. Light is generated when atoms release energy, which in turn produces photons. These photons then scatter in all directions, creating waves of light that travel outward from the source of energy.Step 2: Refraction and ReflectionAs these waves of light travel, they interact with the objects around them, leading to the phenomena of refraction and reflection. When light waves pass through a medium with a different density (such as air and water), they bend, changing direction. This phenomena is known as refraction. Similarly, when light waves hit a smooth, reflective surface, they bounce back, creating a mirror image of the original source.Step 3: AbsorptionNot all the light that is emitted will make it to its final destination. Structures, such as buildings and bodies, can absorb some of the light waves that pass through them. This means that the light arrives at the end of its journey alittle dimmer than when it started.Step 4: The Perception of LightThe final step in this journey is the perception of light. As the waves of light reach our eyes, they activate our retinas, which in turn send a signal to our brain that interprets the image. This is why we are able to see the beauty of a beam of light.In conclusion, a beam of light is a complex, multi-step journey that culminates in a truly stunning experience. From the emission of light to the perception of it by our individual brains, it’s important to appreciate the wonder of this natural phenomena. The next time you see a beam of light, take a moment to appreciate the journey it took to reach your eyes.。

中科院大学张竹青单分子考试总结

一，雷诺数Re（Reynolds number）表示作用于微团的与粘性力[1]之比。

两个几何相似的雷诺数相等，则对应微团的惯性力与粘性力之比相等。

雷诺数较小时，黏滞力对流场的影响大于惯性力，流场中流速的扰动会因黏滞力而衰减，流体流动稳定，为；若雷诺数较大时，惯性力对流场的影响大于黏滞力，流体流动较不稳定，流速的微小变化容易发展、增强，形成紊乱、不规则的流场。

雷诺数越小意味着粘性力影响越显著，越大则惯性力影响越显著。

二，荧光产生原理：光致发光－物质分子吸收光能后，其电子由基态跃迁到激发态，激发态的分子以电磁辐射的形式释放能量回到基态，称为光致发光。

荧光－受光激发的分子从第一激发单重态的最低振动能级回到基态所发出的辐射。

磷光－受光激发的分子从第一激发三重态的最低振动能级回到基态所发出的辐射。

荧光光谱的主要参数和特征：（1）量子产率：荧光量子产率也叫荧光效率或量子效率，它表示物质发射荧光的能力，通常用下式表示发射荧光量子数 / 吸收光量子数（2）荧光寿命：当某种物质被一束激光激发后,该物质的分子吸收后从跃迁到某一激发态上,再以辐射跃迁的形式发出荧光回到基态.当激发停止后,分子的荧光强度降到激发时最大强度的1/e所需的时间称为荧光寿命，它表示粒子在存在的平均时间,通常称为激发态的荧光寿命。

（3）亮度：………斯托克司(stokes)位移：斯托克司位移为最大荧光波长与最大激发波长之差特征：（1）荧光淬灭：是指导致特定物质的荧光强度和寿命减少的所有现象。

（2）荧光漂白：photochemical destruction of a fluorophore, a phenomenon describing a fluorophore inability to be excited again after undergoing repetitive excitation and emission cycles.（3）荧光闪烁：The phenomenon of random switching between ON (bright) and OFF (dark) states of the emitter under its continuous excitation.主要荧光标记：有机染料荧光蛋白量子点三，单分子研究中常用显微术：（原理）（1）激光共聚焦显微镜：Confocal 利用放置在光源后的照明针孔和放置在检测器前的探测针孔实现点照明和点探测，来自光源的光通过照明针孔发射出的光聚焦在样品焦平面的某个点上，该点所发射的荧光成像在探测针孔上，该点以外的任何发射光均被探测针孔阻挡。

光电子单词表中英对照

1. semiconductor: 半导体，常温下导电性能介于导体(conductor)与绝缘体(insulator)之间的材料。

2. light-emitting diode (LED): 发光二极管3. laser diode (LD): 半导体激光器4. photodiode: 光电二极管5. electrons: 电子6. holes: 空穴7. energy gap: 能隙8. photon: 光子9. insulator: 绝缘体10. transistor: 晶体管11. solar cell: 太阳能电池12. quantum dot: 量子点13. doping: 掺杂。

14. Pauli exclusion principle: 泡利不相容原理。

15. Fermi level: 费米能级16. valence band: 价带17. conduction band: 导带18. optical fiber: 光纤19. energy level: 能级。

20. electron–hole pair: 电子-空穴对。

21. impurity: 杂质。

22. dopant: 掺杂剂。

23. intrinsic (pure) semiconductor: 纯半导体。

24. p-type semiconductor: P 型半导体25. n-type semiconductor: N 型半导体。

26. p–n junction: PN 结27. space charge region(depletion layer): 空间电荷区(耗尽层)。

28. forward-bias voltage: 正向偏置电压29. ground state: 基态30. upper level: 上能级31. lower level: 下能级33. electromagnetic radiation:电磁辐射。

爱丁堡仪器 Photoluminescence 光致发光光谱仪微观升级 - MicroPL说明书

Upgrade For Edinburgh Instruments Photoluminescence Spectrometers MicroPLThe MicroPL Upgrade for Edinburgh Instruments Photoluminescence Spectrometers such as the FLS1000 or FS5 allows the study of spectral or time-resolved photoluminescence of samples in the microscopic scale.An Edinburgh Instruments photoluminescence (PL) spectrometer can be converted into a combined spectrometer and microscope system with this user-friendly upgrade.A wide range of microscope configurations, source couplingand detector options are available enabling both steady state and fluorescence lifetime microscopy, as well as automated maps, using the same setup.Just like Edinburgh Instruments spectrometers, the PL microscope is fully configurable to meet your specific needs and can be upgraded with additional capability in the future. It is also possible to couple your own microscope to theFLS1000 or FS5 for a custom MicroPL upgrade (please get in touch for models supported).Key FeaturesEXCITATION SOURCE FLEXIBILITY TO SUIT YOUR APPLICATIONMicroPL is coupled to the spectrometer via liquid light guides or fibres. It is possible to excite the full field of view of the microscope’s objective (widefield excitation), or a specific point in the sample with a laser (point excitation).WIDEFIELD EXCITATIONIn widefield excitation, the spectrometer’s lamp andmonochromator are used to select the wavelength of the light exciting the sample. Wavelength and bandwidth of the excitation light can be set in the software. This provides much more flexibility than traditional microscopy and removes the need for excitation filters – however, an additional white light excitation lamp can be fitted directly to the microscope if desired.POINT EXCITATIONPoint excitation can be performed with either CW or pulsed lasers for spectral or lifetime PL measurements. MicroPL is compatible with Edinburgh Instruments EPL, HPL, and VPL diode lasers. A spot size of ~2 μm (source and objective dependent) is achievable, enabling to resolve PL spectra and lifetimes in the micrometer scale. Both Time-Correlated Single Photon Counting(TCSPC) and Multi-Channel Scaling (MCS) operating modes are compatible, covering a wide range of photoluminescence lifetimes, from a few ps up to seconds. Non-standard applications such as upconversion PL microscopy are possible with theappropriate choice of filters and laser coupling.Widefield microscopy imaging setup (above) and image of BPAE cells labelled with BODIPY , Texas Red and DAPI dyes (below), using FS5 Spectrofluorometer and MicroPL.Point excitation micro-spectroscopy setup (above right), PL spectrum (below) and TCSPC lifetime (below right) of a perovskite sample studied in an FLS1000 Spectrometer with MicroPL upgrade.CameraLaserFromSpectrometer Excitation PathT o SpectrometerEmission PathFLIM ADD-ONMicroPL can be configured with a computer-controlled XYZ stage for Fluorescence Lifetime Imaging Microscopy (FLIM). This upgrade is designed with user-friendliness in mind: you do not need to be a microscopy expert to acquire good quality FLIM data.The FLIM add-on unlocks special features in theFluoracle software including control of the stage and advanced analysis options for maps, such as multi-component decay fitting algorithms.No additional software packages are required to obtain and analyse data.To acquire a FLIM map, the user defines the area or volume to scan across, as well as the spacing between data points and the lifetime acquisition parameters. The software then moves the stage acquiring a fluorescence decay in each data point.The result is a map which can be represented according to intensity, average lifetime or single-component lifetime in each data point, amongst other options. Surface topography and 3D maps can be analysed and displayed in Fluoracle.FLIM MICROSCOPY MADE SIMPLEThe user can define the mapping area and distance between points, view live data to adjust measurement parameters and access a range of map analysis and display options.FLIM map of stained convallaria acquired in FS5 with MicroPL FLIM add-on. Results displayed as colour maps of fluorescence intensity (left) and intensity-weighted average lifetime (right).Registered in England and Wales No: 962331 VAT No:GB 271 7379 37All specifications are correct at the time of production. We reserve the right to change our specifications without notice. ©Edinburgh Instruments Ltd. 2022F / 03.22SpecificationsMANUFACTURED WITH PRIDE IN THE UNITED KINGDOMA MICROSCOPE TAILORED TO YOUR EXACT NEEDSMICROSCOPE MODELSUpright: Nikon NiU, Olympus BX53Inverted: Nikon Ti2-UEXCITATION / EMISSION RANGE 360 nm – 850 nm (std)Can be extended with non-standard UV and NIR objectivesEXCITATION MODESWidefield: tunable continuous source from spectrometer (steady state)Point: EPL/HPL/VPL pulsed lasers (TCSPC or MCS lifetime) and CW lasers (steady state)DETECTION MODESMicro-PL spectroscopy with spectrometerFluorescence Microscopy with additional camera OBJECTIVEMAGNIFICATION Options available from 5X to 100XSAMPLE STAGEManual or PC-controlled XYZ stage with specifications: 75 mm x 50 mm or 130 mm x 85 mm options XY resolution 0.01 µm Z resolution 0.002 µmSOFTWARE Mapping features in Fluoracle included with PC-controlled XYZ stage ACCESSORIESSoftware-controlled cryostat upgradeLight guides coupling to the spectrometer can be configured for the spectral range of interest.Point excitation option adds a laser mount and additional dichroic turret.Widefield excitation allows steady-state spectroscopy with tunable wavelength.White light source for sample visualisation.Sample visualisation camera included. Upgrade to sensitive camera for microscopy images.Binoculars for easier focusing.Dichroic filter cubes customisable to your application.Sample stage can be manual or software controlled.。

爱丁堡仪器FLS980光致发光光谱仪用户手册说明书

Photoluminescence Spectrometer (FLS980)Instrument Summary:The Edinburgh Instruments’ FLS980 photoluminescence spectrometer offers both steady state and time resolved (lifetime) fluorescence spectroscopy measurements of solid and liquid samples. The instrument is equipped with four tunable, pulsed repetition rate lasers (wavelengths including: 315, 375, 635, and 980 nm), a flashlamp, and a xenon arc lamp. The FLS980 incorporates two silicon detectors (200-1100 nm) and an InGaAs detector. By combining time correlated single photon counting (TCSPC) and multi-channel scaling (MCS) the FLS980 excels in fluorescence lifetime measurements which span 12 orders of magnitude, ranging from picoseconds to seconds.Figure 1. The Edinburgh Instruments FLS980, photoluminescence spectrometer.Safety and Precautions:∙Startup: If you are the first user of the day, turn on the FLS980 and let it run for 20 min.∙Shutdown:Turn off the lamp’s and instrument if you are the last user for the day.∙Warning: Avoid running a steady-state excitation or emission scan in which λEx= λEm.∙Warning: Check the compatibility of liquid samples with your cuvettes. Plastic cuvettes (Ex: Polystyrene) can absorb organic liquids (Ex: Toluene) and can leak in the instrument.∙Check:If you do not get signal, check the beam dump to ensure the instrument is configured properly. Additionally, check to make sure there are no optical filters.I. Experiment Planning:1.Determine the Approximate Fluorescence Profiles of your Sample –It is useful to havea basic understanding of the excitation and emission wavelengths of your sample beforestarting an experiment. If the sample has unknown fluorescence properties you can runa signal probing experiment to determine approximate values for excitation and emission.See the “Analysis of Unknown Samples” section below.2.Steady-State Measurement – After determining the approximate excitation and emissionvalues you will want to run a “Steady-State Excitation Scan” and a “Steady-State Emission Scan” to more accurately determine the fluorescence properties of your sample.3.Lifetime Measurement – After determining the steady-state properties of your sample,you are ready to measure the “Fluorescence Lifetime” using either a pulsed LED laser or the flash lamp.II. Start the FLS980:1.Turn on the Instrument –Flip the “PH1” toggle switch behind the computer monitor.2.Turn on the Fan –Flip the “CO1” toggle switch located behind the monitor.3.Turn on Xe Lamp – Flip the lower toggle switch located on the back of the Xe lamp.4.Instrument Stabilization– Allow the instrument to stabilize for 20 minutes.5.Ignite the Xe Lamp– Press and hold the silver button located on top of the instrument.6.Open the Software – Open the Desktop (Password:RandLab). Click the “F980” Icon. III. Insert a Sample:1.Open the Chamber– Lift the circular lid off the top of the FLS980.2.Check Instrument Configuration – Ensure that the beam dump is oriented in the correctposition and that no glass filters are inserted in the path of the beam.3.Insert the Sample in the Holder –The FLS980 can be configured to accommodatecuvettes and films. The mounts are kept in the sample chamber or in the drawers beneath the instrument.*Cuvette Note 1: For liquid samples it is best to utilize dilute concentrations. Samples with high concentrations can limit penetration of the beam through the cuvette. This “inner filter effect” will hurt accuracy of excitation scans. Check to make sure the beam fully penetrates through the liquid.*Cuvette Note 2: Check the compatibility of liquid samples with your cuvettes. Plastic cuvettes (Ex: Polystyrene) can absorb organic liquids and could leak in the instrument.4.Close the Chamber – Replace the lid on the FLS980, this compresses the interlock.5.Position the Sample to Maximize Signal– The sample can be oriented back and forth tomaximize signal by turning the black knob located on the front of the instrument. Check the counts detected in the “Signal Rate Window”.IV. Analysis of Unknown Samples:*Note: For unknown samples it is useful to do a manual excitation and emission scan using the signal rate window. This will provide approximate excitation and emission values prior to running more accurate steady-state excitation and emission scans.1.Open the Signal Rate Window –The “Signal Rate Window” lists live counts as recordedwithin the instrument and can be used to optimize signal parameters (See Figure 2).2.Set the Bandwidth (Δλ) to~0.1–The bandwidth governs the amount variation inwavelength. By setting a low value for unknown samples we prevent detector saturation.3.Set the Step Sizes to ~5 nm – The step size controls the step size taken when scanningthe spectrum. By setting a large step size we can quickly assess the sample.4.Set the Excitation and Emission Wavelengths –Set λEx to ~300 nm and λEm to ~400 nm.5.Manually Increase the Excitation λ–Press the arrow key to iteratively increase theexcitation λ by 5 nm. Monitor the emission signal; look for an increase in CPS. If no signal is detected in this range, increase λEx to ~400 nm and λEm to ~500 nm and continue.6.Scan the Emission λ Range –Once the excitation peak is determined, enter this value asthe excitation wavelength. Next iteratively step through the emission spectrum to find the maximum emission λ.7.Record Approximate Excitation and Emission Wavelengths –These values will helpdesign a steady-state fluorescence procedure.8.Adjust Sample Position – Use the translator knob to adjust sample position and maximizesignal.Figure 2. Screenshot of the signal rate window.*Note: During a steady-state emission scan, the excitation λ is held constant and the detector is used to incrementally scan the light emitted by the sample to determine the emission profile.1.Setup the Emission Scan Parameters– Open the signal rate window and set values.o Excitation Wavelength –Enter an excitation wavelength (λEx), this wavelength (constant) should be at a λ which excites the sample and at least 15 nm shorterthan the onset of the emission profile.o Emission Wavelength –Enter the emission wavelength (λEm), this wavelength should correspond approximately with the emission peak.o Enter Step Size and Band Width –A step size of 0.2 nm and a Δλ of 1.0 should work for most samples.o Select a Light Source and Detector– The Xe900 lamp and the PMT detector should work for most samples.2.Click the “Apply” B utton – This stores the settings in the software.3.Check the Signal – The sample should produce a detectable signal that is between 0 and2 million CPS. If the signal is >2 million CPS, reduce the Iris setting from 100% until thesignal drops below 2 million CPS.4.Open the Steady-State Window –Press the λ icon to open the steady-state scan options.o Check the General Tab– For emission scans select the: EmCorr Scan option.o Check the Excitation Tab–The excitation settings should reflect the settings selected in the signal rate window.5.Update the Emission Tab –Update the “Emission Scan Parameters” options at the bottomof the window.o Scan Range – The scan should begin at a wavelength that is at least 15 nm greater than the λEx value (i.e.λEm Start= λEx + 15 nm) and end at a final value that is ~200nm greater than the starting wavelength (i.e.λEm Stop= λEm Start + 200 nm).o Step – 1.00 nm is a standard step size.o Dwell Time – 0.2 Seconds.o Number of Scans – 5 Scans (this will average data collection over 5 runs).6.Insert a Filter –Add an appropriate high-pass filter that is betweenλEx and λEm Start.o Check for and remove any previous filters.7.Press Start to begin the Scan –The experiment can be aborted by hitting the stop sign.Figure 3. Emission Scan Setup Window.*Note: During a steady-state excitation scan, a constant emission wavelength is monitored to determine CPS produced as the excitation wavelength is varied (See Figure 4).1.Setup the Excitation Scan Parameters– Open the signal rate window and set values.o Excitation Wavelength –Enter an excitation wavelength (λEx), this wavelength should correspond approximately with the excitation peak.o Emission Wavelength –Enter the emission wavelength (λEm), this λshould correspond to a value that is longer than the upper limit of the excitation range.o Enter Step Size and Band Width –A step size of 0.2 nm and a Δλ of 1.0 should work for most samples.o Select a Light Source and Detector – The Xe900 lamp and the PMT detector should work for most samples.2.Click the “Apply” Button – This stores the settings in the software.3.Check the Signal – The sample should produce a detectable signal that is between 0 and2 million CPS. If the signal is >2 million CPS, reduce the Iris setting from 100% until thesignal drops below 2 million CPS.4.Open the Steady-State Window –Press the λ icon to open the steady-state scan options.o Check the General Tab– For excitation scans select: Recording Ref Data.o Check the Emission Tab–The emission settings should reflect the settings selected in the signal rate window.5.Update the Excitation Tab –Update the “E xcitation Scan Parameters” options at thebottom of the window.o Scan Range – The scan should end at a wavelength that is at least ~15 nm less than the emission wavelength value (i.e.λEx Stop= λEm - 15 nm) and start at a value thatis ~200 nm less than the emission wavelength (i.e.λEx Start= λEm - 200 nm).o Step – 1.00 nm is a standard step size.o Dwell Time – 0.2 Seconds.o Number of Scans – 5 Scans (this will average data collection over 5 runs).6.Insert a Filter –Add an appropriate high-pass filter that is betweenλEm and λEx Stop.o Check for and remove any previous filters.7.Press Start to begin the Scan –The experiment can be aborted by hitting the stop sign.Figure 4. Excitation Scan Setup Window.VII. Lifetime Scan:*Note: A lifetime scan can be performed after obtaining the steady-state excitation and emission profile of a sample.1.Configure the Instrument for Lifetime Measurements– Flip the beam dump over to blockthe Xe lamp. Rotate the sample holder 90 ° to face the LED laser source.2.Choose an Appropriate Light Source– Review the excitation and emission data, select alaser closest to the excitation peak of your sample. A range of pulsed LED lasers are available with wavelengths including: 315, 375, 635, and 980 nm. Additionally, a flashlamp is available for samples with long lifetimes.3.Mount the Light Source – Loosen the knob under the current LED source and slide theprevious LED off of the mount. Cap the LED using a red LED end cap. Unplug the red and black cords from the previous LED and connect with the appropriate LED laser. Slide the new LED on the mount and retighten the knob under the LED.4.Turn the LED On – Turn the key on the back of the LED. Wait for the blinking light to stopblinking and stay lit. Then press the red button.5.Select a Repetition Rate – Turn the dial on the LED to a desired repetition rate. This rateshould be long enough to capture the full lifetime of the sample, yet short enough to efficiently collect and bin the data.6.Open the Signal Rate Window – Change the source to the TCSPC Laser. Increase ΔλEm to~5.00 nm. Ensure that the Emission signal is < 2% of the TCSPC’s excitation value. Rotate the optical power knob on the LED to reduce the excitation signal if necessary.7.Open the Lifetime Window– Press the τ icon and select “New Lifetime”.8.Edit Lifetime Settings:o Time Range– This setting should roughly match value selected on the LED dial.o Channels– Set the number of channels (~2048) that you would like to bin data in.Higher values give better resolution but divides counts across more bins.o Stop Condition– Set the Peak counts to value ~2000 counts.9.Press Start – The experiment can take several minutes to hours.10.Check Data– The data should present a peak with a gradually sloping tail. If the data isbinned in such that a series of horizontal lines begin to form, then it is likely that the repetition rate is too high. If this is the case, adjust the knob on the LED and update the Time range setting in the software.*Note: For samples which have extremely fast lifetimes, it may be necessary to deconvolve the instrument’s response function (IRF) from the data. If this is the case, select the “IRF”setting and first run an IRF scan before analyzing your sample.VIII. Data Analysis:bining Spectra–Click the “Join Visible” button at the top of the software. This willcreate a new window that plots multiple spectra on the same graph for comparison.2.Normalizing Data – Data is often normalized for presentation. To normalize data, click“Data”, “Normalize”, select the desired file, and enter a normalized value (Ex: 1.0).3.Data Fitting–Select “Analysis” → “Ex. Tail Fit” → estimate a value for τ1 and select the“Time Resolved Emission Scan (TRES)” option. Alter the τ1 value if it fits the data poorly.4.Saving Data–Export data by selecting “File” and “Export Data to ASCII”.IX. Instrument Shutdown:1.Remove Samples from the Instrument–Remove samples and clean up the instrument.2.Remove Filters from the Instrument – This helps the next user.3.Turn off the Lamp– If you are the last user of the day, turn off the Xe lamp. Allow ~15minutes for the lamp to cool down before powering off the cooling unit.4.Turn off the Fan and Main Power Switch– After the lamp has cooled sufficiently, turn offthe remaining toggle switches in the reverse order as that used to turn on the instrument (turn off CO1 followed by PH1).5.Sign the Log Book.6.Log Off of the Instrument.Emergency Information:Medical Emergencies: Contact 911 and Public Safety (609) 258-1000Room / facility emergencies: Contact Public Safety (609) 258-1000Issues related to the instrument:1. Contact IAC Staff.2.If unsure, leave system as is.3. Try to power down the system.Audible/Siren Emergency Alerts:Follow previous steps 2 & 3 and leave the building.Emergency Contact Information:Nan Yao: Office (609) 258-6394; Cell (908) 922-2236 Email: ******************John Schreiber: Office (609) 258-0034; Cell (215) 431-4670 Email: ******************Paul Shao: Office (609) 258-3851; Cell (847) 721-086 Email: *******************Daniel Gregory: Office (609) 258-7956; Cell (302) 542-3182 Email: ****************************Yao-Wen Yeh: Office (609) 258-7956; Cell (848) 248-8058 Email: ******************。

FS5 Spectrofluorometer brochure

FS5 Fluorescence Spectrometerfrom Single Photons to a Multitude of MeasurementsEnvironmental ScienceManufacturingFood Science and Agriculture• Unique Software, tailored for Fluorescence Spectroscopy• Unique Range in Upgrade Routes, includingº NIR – Extension of Spectral Coverage up to 1650nm º POL – For Measurements of Polarisation and Anisotropy º MCS – Lifetime Measurements in Microsecond to Second Range º TCSPC – Lifetime Measurements in Picosecond to Microsecond Range• Unmatched Range of Measurement ModulesFS5FS5 – Outstanding Instrument SensitivityThe FS5 is designed to meet the highest specifications in the research and analytical markets. From an air-sealed excitation path to enhance the signal in the UV , to performance selected photomultipliers in temperature stabilised housings, from an optical design that includes plane, spherical, toroidal, and ellipsoidal mirrors to fully optimised mirror coatings, the instrument is unique in its spectral performance and sensitivity.Furthermore, the optical design does not only guarantee highest sensitivity and unique wavelength coverage, it has also been optimised for a small bright focus at the sample position. This is important for measuring small sample volumes and benefits many sample holder attachments like plate reader, fibre launch optics, titrators and sample positioners.FS5 – Unique Optical DesignThe FS5 has an optical design that is superior to all known instruments of this class and it uses optical components that are specially selected.The embedded monochromators are of a large focal length (225mm), which ensures better imaging quality and higher optical throughput. The instrument uses a minimum of optical reflections; all optical coatings are ofhighest reflectivity and lowest scatter. We use gratings from the source with the best reputation (Richardson Gratings) and have selected those with highest efficiency and best wavelength coverage.This figure shows typical pulses from a single photon counting photomultiplier.Only photon pulses above a certain threshold value are counted; this eliminates unstable background and associated noise. The amplitude of each accepted pulse is not relevant, a feature of digital data processing.FS5 – Photon CountingSingle Photon Counting is detection at the quantum limit. This detection method is intrinsically digital and therefore less susceptible to noise interference than other methods. As a true counting technique each measured count-value comes with a known statistical accuracy. Therefore the technique is not only the most sensitive, it also offers unique ways in the analysis of your data.Single Photon Counting is employed for all measurement modes, not only for standard spectral scanning and kinetic measurements but also for the optional fluorescence and phosphorescence lifetime measurements.Efficiency curves of the excitation and emission gratings of the FS5.The Raman spectrum of water, exited at 350nm, with a spectral band width of 5nm in excitation and emission, measured with an integration time of 1s, is generally used as a means to specify sensitivity.The FS5 guarantees a signal-to-noise ratio of 4000:1 for these measurement conditions, calculated from the signals taken at the peak at 397nm (b) and the background at 450nm (a) using SNR SQRT = (b-a)/SQRT(a). We can also provide alternative figures which are based on alternative estimates for the noise at 450nm.FS5 – Unique SoftwareThe operating software for the Spectrofluorometer FS5 is Fluoracle, a unique software package specifically written to handle data and instrumentation in fluorescence spectroscopy.Fluoracle is the successor of the proven F980 software. The new Fluoracle controls all Edinburgh Instruments steady state and time resolved spectrometers.The design concept of Fluoracle is straight forward: Focus on all modernphotoluminescence spectroscopy applications, while at the same time provide a user friendly interface with ‘ready to publish’ outputs.Whether you select a basic spectral scanning version of the FS5 or you go for an advanced version that includes TCSPC lifetime measurements, the software will include all instrument options, including data acquisition, analysis and presentation.FS5 – Reliability and AccuracyThorough calibration procedures are used for spectral calibration (wavelength accuracy and repeatability) and photometric calibration (presentation of true spectra without distortion of instrumental throughput functions).We use traceable standards for calibration throughout the full wavelength coverage. We will verify calibration, in photons per unit bandwidth, by NIST and BAM standards. The FS5 delivers data you can trust.NIST standards (top) and BAM standards (bottom), as measured with the FS5 under recommended measurement conditions.Load / Save Measurement Settings ✓Batch Measurements ✓Detailed Measurement Properties ✓Higher Order Removal ✓ASCII Input / Output ✓Recognition of Sample Holders / Accessories ✓Chromaticity Analysis ✓Quantum Yield Analysis ✓Reflection / Absorption✓Synchronous Scans - with concentration dependenceIn synchronous spectral scans the excitation and emission monochromators scan at the same time with a fixed wavelength offset. For dilute mixtures this type of scan is used to identify species with a strong overlap between absorption and emission.Synchronous scans, together with the integrating sphere attachment, can also be used to measure the absorption spectra of strongly scattering powders.YAG: Ce powder, diluted with BaSO 4 to study the effect of re-absorption/emission, concentration change from 100% down to 20%. The software wizard is used to calculatethe Absorbance (top left) from the raw data of synchronous scans (bottom right).Absorption Scans / Kinetic ScansThe FS5 can record the time course of a fluorescence signal, and – at the same time – record the signal transmitted through the sample. This enables experiments to be performed with chemically or biologically unstable samples, or with samples where very small changes are to be measured very accurately.The transmission detector is standard in the FS5.Caspase Assay, fluorescence time course recorded for a 100% enzyme addition (blue) and a 0% enzyme control (red). Peptide cleavage is recorded by an organic dye excited at 400nm, emitting at 460nm.Excitation Scans - with pH dependenceFluorescence excitation spectra are more selective than absorption spectra, as they reveal – by virtue of the selected emission wavelength – the absorption of a particular emitting species.Accurate excitation spectra require a sensitive instrument, as the concentration of the sample must be kept low to avoid inner filter effects, and require reliable spectral correction, as the xenon lamp has narrow features at certain wavelengths.Fluorescein in water , with pH adjusted between pH2 and pH7Spectral Band width: 1.5nm, dwell time: 0.1spH adjusted between pH2 (blue shifted spectrum) and pH7 (spectrum of maximum intensity)Measurements of absolute fluorescence quantum yieldFluorescence quantum yields can be measured by using the optional integrating sphere. The absolute method requires two measurements of the number of absorbed photons and the number of the emitted photons. The number of absorbed photons of a sample is determined by the reduction of the light scatter compared to a blank measurement.The quantum yield calculation is made using a wizard within the operating software.Plate ReaderMultiple sample measurements can be made using the plate reader sample holder accessory. This can be used for liquid samples with commercial plates of up to 96 wells, but is also suitable for routine quality assessment of fluorescent powders. As with all the sample holder accessories this is also compatible with the FS5 upgrade options, including the lifetime upgrades.Excitation-Emission MapsExcitation-Emission Maps (EEMs) provide a ‘Finger Print’ of complex mixtures of substances. These maps can be measured either by a series of emission scans with stepwise increase, or decrease of the excitation wavelength or by a series of synchronous scans and stepwise increase of the excitation-emission offset.A map measurement over a wide range of excitation and emission wavelengths, as shown here, can only be performed properly if higher order scatter is automatically removed during the measurement.Chinese Green Tea (Wuyi region).Spectral Band width: 5nm, step size: 2nm, dwell time: 0.1sfor the determination of colour co-ordinates and luminocity values using CIE 1931 and CIE Quinine bisulphate in Perchloric acid.The red curve shows the scan over the excitation scatter at 350nm and the emission of the sample, the blue curve shows the scatter of the blank measurement. The scatter region has been scaled by a factor 100 for better demonstration.Quality control measurement of Y 2O 3 Eu 3+ powder samples, in a 12 well powder tray. The graph shows 12 superimposed identical measurements. The inset is an example of the measurement progress display.FS5 – NIR Extension of the Spectral Coverageinto the Near InfraredThis is a unique upgrade route that is only available to the FS5. The FS5-NIR has a SECOND detector fitted to expand the operating spectral range without sacrificing the performance of the standard instrument.There are two NIR versions available: FS5-NIR is fitted with an extra cooled side window PMT and selected grating for sensitivity up to 1100nm; FS5-NIR+ is fitted with a TE cooled NIR-PMT and NIR-grating for sensitivity up to 1650nm.Both options are based on single photon counting for maximum sensitivity and compatibility with any of the lifetime options, should they be added.FS5 – POL Measurements of FluorescencePolarisation and AnisotropyThis upgrade comprises the standard FS5 with fully computerised polarisers in both excitation and emission arms. The polarisers enable polarised fluorescence and fluorescence anisotropy studies.Automated measurements and generation of anisotropy curves, both raw and G-factor corrected, are supported by the software. If combined with the TCSPC option, time resolved fluorescence anisotropy measurements and analysis will also be possible.FS5-POL uses a BBO polarising prisms with an operational range of 250nm-900nm for both excitation and emission. This version is therefore compatible with the standard detector of the FS5. An option is available to replace the polarising prism of the emission beam path by a Calcite prism with an operational range 300nm to >1650nm. This ensures compatibility with the N IR options FS5-NIR and FS5-NIR+.All the standard features of the FS5 are retained when an Upgrade Option has been added. Many of the options can be combined.This offers outstanding flexibility for an instrument of this class.Fluorescence Anisotropy measurement of Rhodamin 6G in glycerol at room tem-perature.Raw data for vertical (red) and horizontal (green) emis-sion polarisa-tion plane.Steady state anisotropy of excitation (blue) and emission (magenta)Measurement of the emission spectrum from an infrared laser dye with standard FS5 photomultiplier (blue) and with the FS5-NIR (brown)The emission spectrum of Nd:YAG, meas-ured with the FS5-NIR+The instrument has all the features of the standard FS5, plus the capability of long lifetime (>10m s) measurements for the time resolved measurement of strong phosphors and rare earth emissions.The changeover between the standard continuous light source and the pulsed xenon flash lamp is software controlled and the acquisition mode automatically changes from standard photon counting to time resolved photon counting. The software incorporates fitting and reconvolution analysis for lifetime evaluation.For longer working sessions in lifetime mode, the continuous lamp can be switched off via the spectrometer software as it is not required. This saves energy and increases the lifespan of this lamp.The instrumental response width of this instrument is 2m s. Lifetimes from below 10m s to above 10s can be accurately measured.FS5 – TCSPC Measurement ofLifetimes in Picosecond to Microsecond RangeThe instrument has all the features of the standard FS5, plus the capability of fluorescence lifetime measurements in the picosecond, nanosecond and the lower microsecond (<10m s) time range. The FS5-TCSPC version requires picosecond pulsed diode lasers and LEDs for excitation, which are simply attached to the special FS5-TCSPC housing and are compatible to all sample holder options.There is no stand-alone laser driver or data acquisition module! The software is fully compatible with all measurement options and offers numerical reconvolution and curve fitting.Note that the picosecond diode lasers (EPL series) and the picosecond pulsed LEDs (EPLED series) come with different output wavelengths. More than one of these picosecond pulsed light sources may be required to cover your range of applications.TCSPC lifetime measurements with the standard FS5 detector will have an instrumental response width of ~800ps (FS5-TCSPC ). The exact value depends on which EPL or EPLED model is used. For challenging applications the instrumental response width can be improved by fitting a SECOND, faster detector (FS5-TCSPC+). Using an EPL as an excitation source in this configuration will result in an instrumental response width of ~250ps.MCS measurements in the microsecond andmillisecond time scale on an example immune-assay.In this assay, the FRET rate of the donor-acceptor pair (Europium cryptate-APC) used to label the antibodies depends on the concentration of the antigen. Very low antigen concentrations can be measured due to the discrimination by lifetimes.Measurement example for theFS5-TCSPC upgrade, using an EPLED 280 and the standard detector. (Sample: Norharmane in ethanol, atdifferent emission wavelengths.)Measurement example for the FS5-TCSPC+upgrade, with EPL 405 and faster detector. (Sample: Hematoporphyrine in PBS, at different emission wavelength.)The FS5-TCSPC, with EPL / EPLED sources.Measurement ModulesSC-80 Cryostat CassetteSC-60 Calibration KitSC-25 TE Cooled Sample HolderSC-05 Standard Cuvette HolderSC-20 Cuvette Holder with Temp Sensor for Coolant CirculationSC-10 FF Holder for Cuvettes, Powders and FilmsSC-15 FF Holder (Vertical) for Powders and FilmsMeasurement modules are available for practically all applications.These accessories can be purchased at any time and can be easily installed by the user. The installation takes no which of the measurement modules is in use. This reduces complexity in the user interface and ensures SC-50 Remote Fibre InterfaceSC-40 Plate ReaderSC-30 Integrating SphereSC-70 Liquid Nitrogen DewarWavelength Accuracy – Excitation/Emission:FS5 Fluorescence SpectrometerFor more information contact:Edinburgh Instruments Ltd。

时间分辨荧光寿命英文缩写

时间分辨荧光寿命英文缩写Time-resolved fluorescence lifetime (TRFL) is a technique used in spectroscopy to measure the decay time of fluorescence after excitation. It provides valuable information about the molecular environment and interactions of fluorophores. TRFL measurements are commonly used in various scientific fields, such as biology, chemistry, material science, and medical diagnostics.The most commonly used method for TRFL measurements is time-correlated single photon counting (TCSPC). In TCSPC, a pulsed laser is used to excite the sample, and the resulting fluorescence emission is collected and detected using a photon-counting detector. The detector records the arrival time of each emitted photon relative to the excitation pulse, and this information is used to build a decay curve. The decay curve represents the fluorescence intensity as a function of time, and the fluorescence lifetime can be derived from the decay curve.The fluorescence lifetime is the average time that a fluorophore spends in the excited state before returning to the ground state. It is determined by various factors, including the molecular structure, solvent environment, and the presence of other molecules that can quench or enhance the fluorescence. By measuring the fluorescence lifetime, researchers can obtain valuable information about the structure and dynamics of molecules, as well as their interactions with other molecules.TRFL measurements have been widely used in the study of biological systems. For example, in fluorescence microscopy, TRFL can be used to differentiate between different fluorophoresin a sample based on their fluorescence lifetimes. This allows for the simultaneous detection of multiple fluorophores with overlapping emission spectra. TRFL can also be used to study protein-protein interactions, DNA-protein interactions, and membrane dynamics in living cells.In the field of chemistry, TRFL can be used to study the kinetics and mechanisms of chemical reactions. By monitoring changes in fluorescence lifetime during a reaction, researchers can gain insights into reaction intermediates and transition states. TRFL can also be used to study the properties of nanomaterials, such as quantum dots and nanoparticles, as well as the behavior of dyes and sensors.In medical diagnostics, TRFL has been used in various applications, such as drug discovery, clinical diagnostics, and imaging. For example, TRFL-based assays can be used to detect and quantify specific biomarkers in clinical samples, such as blood or urine, for disease diagnosis and monitoring. TRFL imaging techniques, such as fluorescence lifetime imaging microscopy (FLIM), can provide high-resolution images of biological samples, allowing for the visualization of molecular interactions and spatial localization of fluorophores.In summary, TRFL is a powerful technique for studying the fluorescence properties of molecules. It provides valuable information about the molecular environment, interactions, and dynamics. TRFL has applications in various scientific fields, including biology, chemistry, material science, and medicaldiagnostics, and it continues to contribute to advancements in these areas.。

与激光有关的英文文献

与激光有关的英文文献Revised at 16:25 am on June 10, 2019L a s e r t e c h n o l o g y R. E. Slusher Bell Laboratories, Lucent Technologies, Murray Hill, New Jersey 07974 Laser technology during the 20th century is reviewed emphasizing the laser’s evolution from science to technology and subsequent contributions of laser technology to science. As the century draws to a close, lasers are making strong contributions to communications, materials processing, data storage, image recording, medicine, and defense. Examples from these areas demonstrate the stunning impact of laser light on our society. Laser advances are helping to generate new science as illustrated by several examples in physics and biology. Free-electron lasers used for materials processing and laser accelerators are described as developing laser technologies for the next century.S0034-68619902802-01. INTRODUCTIONLight has always played a central role in the study of physics, chemistry, and biology. Light is key to both the evolution of the universe and to the evolution of life on earth. This century a new form of light, laser light, has been discovered on our small planet and is already facilitating a global information transformation as well as providing important contributions to medicine, industrial material processing, data storage, printing, and defense. This review will trace the developments in science and technology that led to the invention of the laser and give a few examples of how lasers are contributing to both technological applications and progress in basic science. There are many other excellent sources that cover various aspects of the lasers and laser technology including articles from the 25th anniversary of the laser Ausubell and Langford, 1987 and textbooks ., Siegman, 1986; Agrawal and Dutta, 1993; and Ready, 1997.Light amplification by stimulated emission of radiation LASER is achieved by exciting the electronic, vibrational, rotational, or cooperative modes of a material into a nonequilibrium state so that photons propagating through the system are amplified coherently by stimulated emission. Excitation of this optical gain medium can be accomplished by using optical radiation, electrical current and discharges, or chemical reactions. The amplifying medium is placed in an optical resonator structure, for example between two high reflectivity mirrors in a Fabry-Perot interferometer configuration. When the gain in photon number for an optical mode of the cavity resonator exceeds the cavity loss, as well as loss from nonradiative and absorption processes, the coherent state amplitude of the mode increases to a levelwhere the mean photon number in the mode is larger than one. At pump levels above this threshold condition,the system is lasing and stimulated emission dominates spontaneous emission. A laser beam is typically coupled out of the resonator by a partially transmitting mirror. The wonderfully useful properties of laser radiation include spatial coherence, narrow spectral emission, high power, and well-defined spatial modes so that the beam can be focused to a diffraction-limited spot size in order to achieve very high intensity. The high efficiency of laser light generation is important in many applications that require low power input and a minimum of heat generation.When a coherent state laser beam is detected using photon-counting techniques, the photon count distribution in time is Poissonian. For example, an audio output from a high efficiency photomultiplier detecting a laser field sounds like rain in a steady downpour. This laser noise can be modified in special cases, ., by constant current pumping of a diode laser toobtain a squeezed number state where the detected photons sound more like a machine gun than rain. An optical amplifier is achieved if the gain medium is not in a resonant cavity. Optical amplifiers can achievevery high gain and low noise. In fact they presently have noise figures within a few dB of the 3 dB quantum noise limit for a phase-insensitive linear amplifier, ., they add little more than a factor of two to the noise power of an input signal. Optical parametric amplifiers OPAs, where signal gain is achieved by nonlinear coupling of a pump field with signal modes, can be configured to add less than 3 dB of noise to an input signal. In an OPA the noise added to the input signal can be dominated by pump noise and the noise contributed by a laser pump beam can be negligibly small compared to the large amplitude of the pump field.2. HISTORYEinstein 1917 provided the first essential idea for the laser, stimulated emission. Why wasn’t the laser invented earlier in the century Much of the early work on stimulated emission concentrates on systems near equilibrium, and the laser is a highly nonequilibrium system. In retrospect the laser could easily have been conceived and demonstrated using a gas discharge during the period of intense spectroscopic studies from 1925 to 1940. However, it took the microwave technology developed during World War II to create the atmosphere for thelaser concept. Charles Townes and his group at Columbia conceived the maser microwave amplification by stimulated emission of radiation idea, based on their background in microwave technology and their interest in high-resolution microwave spectroscopy. Similar maser ideas evolved in Moscow Basov and Prokhorov, 1954 and at the University of Maryland Weber, 1953. The first experimentally demonstrated maser at Columbia University Gordon et al., 1954, 1955 was based on an ammonia molecular beam. Bloembergen’s ideas for gain in three level systems resulted in the first practical maser amplifiers in the ruby system. These devices have noise figures very close to the quantum limit and were used by Penzias and Wilson in the discovery of the cosmic background radiation.Townes was confident that the maser concept could be extended to the optical region Townes, 1995. The laser idea was born Schawlow and Townes, 1958 when he discussed the idea with Arthur Schawlow, who understood that the resonator modes of a Fabry-Perot interferometer could reduce the number of modes interacting with the gain material in order to achieve high gain for an individual mode. The first laser was demonstrated in a flash lamp pumped ruby crystal by Ted Maiman at Hughes Research Laboratories Maiman, 1960. Shortly after the demonstration of pulsed crystal lasers, a continuouswave CW He:Ne gas discharge laser was demonstrated at Bell Laboratories Javan et al., 1961, first at mm and later at the red nm wavelength lasing transition. An excellent article on the birth of the laser is published in a special issue of Physics Today Bromberg, 1988.The maser and laser initiated the field of quantum electronics that spans the disciplines of physics and electrical engineering. For physicists who thought primarilyin terms of photons, some laser concepts were difficult to understand without the coherent wave concepts familiar in the electrical engineering community. For example, the laser linewidth can be much narrower than the limit that one might think to be imposed by the laser transition spontaneous lifetime. Charles Townes won a bottle of scotch over this point from a colleague at Columbia. The laser and maser also beautifully demonstrate the interchange of ideas and impetus between industry, government, and university research.Initially, during the period from 1961 to 1975 there were few applications for the laser. It was a solution looking for a problem. Since the mid-1970s there has been an explosive growth of laser technology for industrial applications. As a result of this technology growth, a new generation of lasers including semiconductor diode lasers, dye lasers, ultrafast mode-locked Ti:sapphire lasers, optical parameter oscillators, and parametric amplifiers is presently facilitating new research breakthroughs in physics, chemistry, and biology.3. LASERS AT THE TURN OF THE CENTURYSchawlow’s ‘‘law’’ states that everything lases if pumped hard enough. Indeed thousands of materials have been demonstrated as lasers and optical amplifiers resulting in a large range of laser sizes, wavelengths, pulse lengths, and powers. Laser wavelengths range from the far infrared to the x-ray region. Laser light pulses as short as a few femtoseconds are available for research on materials dynamics. Peak powers in the petawatt range are now being achieved by amplification of femtosecond pulses. When these power levels are focused into a diffraction-limited spot, the intensities approach 1023 W/cm2. Electrons in these intense fields are accelerated into the relativistic range during a single optical cycle, and interesting quantum electrodynamic effects can be studied. The physics of ultrashort laser pulses is reviewed is this centennial series Bloembergen, 1999.A recent example of a large, powerful laser is the chemical laser based on an iodine transition at a wavelength of mm that is envisioned as a defensive weapon Forden, 1997. It could be mounted in a Boeing 747 aircraft and would produce average powers of 3 megawatts, equivalent to 30 acetylene torches. New advances in high quality dielectric mirrors and deformable mirrors allow this intense beam to be focused reliably on a small missile carrying biological or chemical agents and destroy it from distances of up to 100 km. This ‘‘star wars’’ attack can be accomplished during the launch phase of the target missile so that portions of the destroyed missile would fall back on its launcher, quite a good deterrent for these evil weapons. Captain Kirk and the starship Enterprise may be using this one on the Klingons At the opposite end of the laser size range are microlasers so small that only a few optical modes are contained in a resonator with a volume in the femtoliter range. These resonators can take the form of rings or disks only a few microns in diameter that use total internal reflection instead of conventional dielectric stack mirrors in order to obtain high reflectivity. Fabry-Perot cavities only a fraction of a micron in length are used for VCSELs vertical cavity surface emitting lasers that generate high quality optical beams that can be efficiently coupled to optical fibers Choquette and Hou, 1997. VCSELs may find widespread application in optical data links.4. MATERIALS PROCESSING AND LITHOGRAPHYHigh power CO2 and Nd:YAG lasers are used for a wide variety of engraving, cutting, welding, soldering, and 3D prototyping applications. rf-excited, sealed off CO2 lasers are commercially available that have output powers in the 10 to 600 W range and have lifetimes of over 10 000 hours. Laser cutting applications include sailclothes, parachutes, textiles, airbags, and lace. The cutting is very quick, accurate, there is no edge discoloration, and a clean fused edge is obtained that eliminatesfraying of the material. Complex designs are engraved in wood, glass, acrylic, rubber stamps, printing plates, plexiglass, signs, gaskets, and paper. Threedimensional models are quickly made from plastic or wood using a CAD computer-aided design computer file.Fiber lasers Rossi, 1997 are a recent addition to the materials processing field. The first fiber lasers were demonstrated at Bell Laboratories using crystal fibers in an effort to develop lasers for undersea lightwave communications. Doped fused silica fiber lasers were soon developed. During the late 1980s researchers at Polaroid Corp. and at the University of Southampton invented cladding-pumped fiber lasers. The glass surrounding the guiding core in these lasers serves both to guide the light in the single mode core and as a multimode conduit for pump light whose propagation is confined to the inner cladding by a low-refractive index outer polymer cladding. Typical operation schemes at present use a multimode 20 W diode laser bar that couples efficiently into the large diameter inner cladding region and is absorbed by the doped core region over its entire length typically 50 m. The dopants in the core of the fiber that provide the gain can be erbium for the mm wavelength region or ytterbium for the mm region. High quality cavity mirrors are deposited directly on the ends of the fiber. These fiber lasers are extremely efficient, with overall efficiencies as high as 60%. The beam quality and delivery efficiency is excellent since the output is formed as the single mode output of the fiber. These lasers now have output powers in the 10 to 40 W range and lifetimes of nearly 5000 hours. Current applications of these lasers include annealing micromechanical components, cutting of 25 to 50 mm thick stainless steel parts, selective soldering and welding of intricate mechanical parts, marking plastic and metal components, and printing applications.Excimer lasers are beginning to play a key role in photolithography used to fabricate VLSI very large scale integrated circuit chips. As the IC integrated circuit design rules decrease from mm 1995 to mm 2002, the wavelength of the light source used for photolithographic patterning must correspondingly decrease from 400 nm to below 200 nm. During the early 1990s mercury arc radiation produced enough power at sufficiently short wavelengths of 436 nm and 365 nm for high production rates of IC devices patterned to mm and mm design rules respectively. As the century closes excimer laser sources with average output powers in the 200 W range are replacing the mercury arcs. The excimer laser linewidths are broad enough to prevent speckle pattern formation, yet narrow enough, less than 2 nm wavelength width, to avoid major problems with dispersion in optical imaging. The krypton fluoride KF excimer laser radiation at 248 nm wavelength supports mm design rules and the ArF laser transition at 193nm will probably be used beginning with mm design rules. At even smaller design rules, down to mm by 2008, the F2 excimer laser wavelength at 157 nm is a possible candidate, although there are no photoresists developed for this wavelength at present. Higher harmonics of solid-state lasers are also possibilities as high power UV sources. At even shorter wavelengths it is very difficult for optical elements and photoresists to meet the requirementsin the lithographic systems. Electron beams, x-rays and synchrotron radiation are still being considered for the 70 nm design rules anticipated for 2010 and beyond.5. LASERS IN PHYSICSLaser technology has stimulated a renaissance in spectroscopies throughout the electromagnetic spectrum. The narrow laser linewidth, large powers, short pulses, and broad range of wavelengths has allowed new dynamic and spectral studies of gases, plasmas, glasses, crystals, and liquids. For example, Raman scattering studies of phonons, magnons, plasmons, rotons, and excitations in 2D electron gases have flourished since the invention of the laser. Nonlinear laser spectroscopies have resulted in great increases in precision measurement as described in an article in this volume Ha¨nsch and Walther 1999.Frequency-stabilized dye lasers and diode lasers precisely tuned to atomic transitions have resulted in ultracold atoms and Bose-Einstein condensates, also described in this volume Wieman et al., 1999. Atomicstate control and measurements of atomic parity nonconservation have reached a precision that allows tests of the standard model in particle physics as well as crucial searches for new physics beyond the standard model. In recent parity nonconservation experiments Wood et al., 1997 Ce atoms are prepared in specific electronic states as they pass through two red diode laser beams. These prepared atoms then enter an optical cavity resonator where the atoms are excited to a higher energy level by high-intensity green light injected into the cavity from a frequency-stabilized dye laser. Applied electric and magnetic fields in this excitation region can be reversed to create a mirrored environment for the atoms. After the atom exits the excitation region, the atom excitation rate is measured by a third red diode laser. Very small changes in this excitation rate with a mirroring of the applied electric and magnetic fields indicate parity nonconservation. The accuracy of the parity nonconservation measurement has evolved over several decades to a level of %. This measurement accuracy corresponds to the first definitive isolation of nuclear-spin-dependent atomic parity violation.。

核临界和辐射防护术语中英文对照

[Particle] fluence rate Energy fluence rate Particle radiance Energy radiance Energy imparted Linear energy Specific energy[imparted] Absorbed dose Absorbed dose rate kerma Kerma rate Exposure Exposure rate Decay constant Radioactive half-life Biological half-life Effective half-life Activity Surface activity Activity concentration Specific activity Air kerma rate constant Dose equicalent Dose equivalent Dose equivalent rate Adsorbed dose index Quality factor Dose equivalent indx Surface dose equivalent index Depth dose equivalent index Cross section Lethargy Attenuation coefficient Mass attenuation coefficient Mass energy transfer coefficient adsorption coefficient Mass energy adsorption coefficient Total mass stopping coefficient Linear energy transfer, LET
核临界术语临界的，临界中子反照率吸收剂量中子吸收临界事故报警系统 α 粒子 β 粒子曲率瞬发脉冲核闪变周期（T）指数柱体照射量几何良好易裂变核素易裂变系统核裂变裂变产物核闪变的裂变产额可裂变核素 γ 辐射戈瑞危险倒时数致电离辐射 “辐照” 辐射反应性非补偿反应性反射层反射层节省相对生物效应雷姆雷普危险度伦琴紧急停堆停堆机制 Critical,Criticality Albedo,neutron Absorbed dose Absorption,neutron Alarm system,criticality accident Alpha particle Beta particle buckling Burst,prompt Excursion period Exponential column exposure Favorable geometry Fissile nucleus Fissile system Fission,nuclear Fission products Fissionyield,excursion Fissionable nucleus Gamma radiation Gray (Gy) hazard inhour Ionizing radiation irradiation radiation reactivity Reactivity,uncompensated reflector Reflector savings (RBE) rem rep risk roentgen scram Shutdown mechanism

放射学实践

《放射学实践》杂志中文名称：放射学实践外文名称：Radiologic Practice语言：中文类别：出版，医学期刊主管单位：中华人民共和国卫生部主办单位：华中科技大学同济医学院编辑单位：《放射学实践》编辑部创刊时间：1986出版周期：月刊国内刊号CN：42-1208/R国际刊号ISSN：1000-0313邮发代号：38-122《放射学实践》杂志简介《放射学实践》1986年创刊,经国家科委批准[(85)国科发策字738号],由中华人民共和国卫生部主管,同济医科大学主办的国家级影像学学术期刊,由国内著名影像专家郭俊渊教授担任主编.起初是与德国Hirzel出版社合作出版,是国内唯一一本与德国合作出版的影像医学专业期刊.发行遍及范围中国、德国、台湾、及香港等国家和地区。

自创刊以来，《放射学实践》以其内容新颖、学术价值高、翻译准确、文笔流畅、印刷精美广受欢迎，为我国影像医学的发展及引进德国先进影像技术等作出了巨大贡献，成为我国中文科技核心期刊之一。

随着我国影像医学的迅猛发展，《放射学实践》在创刊后第10年即1996年扩大和改组了编委会，开始刊登国内专家学者的优秀文章。

及时传递最新适用的医学影像信息，深受作者和学者的好评，取得了很大的社会效益和经济效益。

该刊创刊24年来一直关注国内外放射学方面的新进展、新动态和新技术。

全面介绍x线、CT、MRI、介入放射学和放射治疗、核医学等影像医学方面的新知识、新经验，既有理论介绍，又有实践经验交流。

内容全面、新颖，刊登的文章均有较强的学术性、科学性和实用性，对读者有较大的指导性和借鉴价值，引导着医学影像学的发展，为促进我国的影像医学发展起到了重要的作用，是专业内影响最大的学术期刊之一。

该刊封面彩色印刷，平行向上四边形的一角托放文稿中最先进的影像图片的设计，寓含着本刊和诸国医学前进的脚步-与最先进的影像医学平行发展、同步前进。

黑、绿、蓝象征着"现代、生命、激情、奉献"的色彩，呈现图像完美品质，传递信息准确内涵，深受影像工作者的喜爱。

Fluorescence Resonance Energy Transfer (FRET)荧光共振能量转移(FRET)

(H3C)2N
• chemical attachment of synthetic dyes
O
N+(CH3)2
OCH3 O
R
Absorption / Emission (a.u.)
580
fluorescence
a typical synthetic chromophore: tetramethylrhodamine
#emitted photons·molecule-1·s-1
FRET
488nm light
excitation
excitation
FITC
FITC 520nm light
TRITC
TRITC
630nm light
FRET
Distance dependent interaction between the electronic excited states of two molecules *not sensitive to the surrounding solvent shell of a
Absorption Emission
400 450 500 550 600 650 700 wavelength (nm)
• extinction coefficient: ~100,000 Molar-1 cm-1 • fluorescence quantum yield: ~50% • triplet quantum yield <1% • available in reactive forms (to attach to amines,
NAD(P)H
R
are not good enough
NH

Fluorescence Recovery After Photobleaching (FRAP 荧光漂白后恢复(FRAP

Problems with FRAP of cytoplasmic components (2 orders of magnitude faster
than membranes)
1. Diffusion is fast compared to bleaching and monitoring rate D=ms : cannot truly scan
Spot Photobleaching
•Bleach and monitor single diffraction limited spot •Assumes infinite reservoir of fluorescent molecules (hole can fill back in) •Use D = w2/4D to obtain D •Determine w = nominal width of Gaussian spot by other optical method 1/e2 point •Fit fluorescence recovery curve to obtain D
Real FRAP data
More Diffusion types
Fx,t/F()12texpxt2/w2t Fullyrecovers
with
w2tw021t/D at/1t/
Important for Large macromolecules: Collisions, obstacles, binding
2 If use small bleach regions, redistribution may occur during bleaching. In fact, often cannot observe bleach of small region at all.

Appl.Phys.Lett.,1998,1637

Time resolved photoluminescence investigations of polyacetylene derivativesHui Wang a)Department of Physics,Zhongshan University,Guangzhou510275,People’s Republic of ChinaKam Sam WongDepartment of Physics,Hong Kong University of Science and Technology,Clear Water Bay,Kowloon,Hong Kong͑Received11March1998;accepted for publication23June1998͒Time resolved photoluminescence dynamics of poly͑phenylacetylene͒͑PPA͒andpoly͑1-phenyl-1-butyl͒͑PPB͒were investigated by using femtosecond luminescence upconversionspectroscopy technique.An ultrafast initial luminescence decay͑ϳ5ps͒was observed for PPA,butit was absent for PPB.The transient luminescence spectrum of PPA exhibits a much larger red-shift ͑ϳ140meV͒than that of PPB͑ϳ60meV͒.PPB shows a much higher luminescence efﬁciency than that of PPA.The inﬂuence of the substitution on the photoluminescence behaviors of PPA and PPBis discussed.©1998American Institute of Physics.͓S0003-6951͑98͒02734-X͔In recent years,photoluminescence͑PL͒and electrolu-minescence͑EL͒properties of conjugated polymers have at-tracted much attentions because of their promising applica-tions in optical and optoelectronic devices.1,2Polyacetylenederivatives represent a big family of conjugated polymersand have substantially different properties from those ofpolyacetylene.For example,they are fairly stable in air andhighly transparent because of their large energy band gaps.3Recently,some polyacetylene derivatives such as poly ͑diphenylacetylene͒͑PDPA͒have shown high PL and EL ef-ﬁciencies,which are comparable to those of the well-studiedEL materials such as poly͑p-phenylene vinylene͒͑PPV͒and8-hydroxy quinoline aluminum͑Alq3͒.4Since various sub-stitutes can be attached onto the main backbone,a largenumber of polyacetylene derivatives can be derived for theapplications of optoelectronic devices.Preliminary studieshave shown that the substitutes have an important inﬂuenceon the PL and EL efﬁciencies of polyacetylene derivatives.Monosubstituted polyacetylenes such as poly͑phenylacetyle-ne͒͑PPA͒show low PL and EL efﬁciencies.However,disubstituted polyacetylenes such as PDPA usually exhibitmuch higher PL and EL efﬁciencies.4In order to explain thedifference of luminescence͑PL and EL͒properties observedin these polyacetylene derivatives,the understanding of therecombination mechanisms and their dependence on the sub-stitution of polyacetylene backbone is fundamentally impor-tant.We have therefore performed detail investigations on the time resolved photoluminescence of PPA and poly͑1-phenyl-1-butyl͒͑PPB͒.PPA and PPB powders were synthesized using a group of new organorhodium catalysts from actylene as a monomer.5,6The products of polymerization were rich in cis-isomer͑94%͒.The molecular structures of PPA and PPB are depicted in Figs.1͑a͒and1͑b͒,respectively.Both PPA and PPB molecules under study were dissolved in toluene with the concentration of about20mg/ml.The time resolved PL dynamics of PPA and PPB are studied by using femtosecond luminescence upconversion spectroscopy technique.7The laser source is a Kerr–Lens–Modelocked Ti-sapphire laser with chirped pulse ampliﬁca-tion,which produces150fs laser pulse with the photon en-ergy of1.6eV and a repetition rate of1kHz.The sample solution is of1mm thick and excited by frequency-doubled laser pulses with the photon energy of about3.2eV and the excitation power is about300␮W.All the measurements were performed at roomtemperature.FIG.1.Molecular structure of the materials studied:͑a͒poly͑phenylacety-lene͒͑PPA͒;͑b͒poly͑1-phenyl-1-butyl͒͑PPB͒.a͒Electronic mail:wang-hui@FIG.2.Steady-state photoluminescence spectra of͑a͒PPA and͑b͒PPBexcited at3.2eV.APPLIED PHYSICS LETTERS VOLUME73,NUMBER1221SEPTEMBER199816370003-6951/98/73(12)/1637/3/$15.00©1998American Institute of PhysicsFigure 2shows the steady-state ͑PL ͒spectra of PPA and PPB excited by the photon energy of 3.2eV,which is coin-cident with the excitation photon energy in the transient ex-periments.PPB shows blue-green light emission with a peak around 2.7eV,which is much efﬁcient than that of PPA at 2.5eV.The color change from blue-green to green is due to the decrease of the length of butyl side chain.Similar results have been reported in Ref.8.Time resolved PL spectra of PPA and PPB recorded at different time delays are presented in Figs.3͑a ͒and 3͑b ͒,respectively.Both PPA and PPB show the instantaneous lu-minescence,reﬂecting the ultrafast vibronic relaxation on a time scale much shorter than the time resolution of the setup.The transient spectra of PPA and PPB evolve rapidly to the shapes similar to the steady-state luminescence in the ﬁrst few picoseconds,accompanying signiﬁcant red-shifts of the transient luminescence spectra.For comparison,the peak po-sitions of the transient luminescence spectra versus time for both PPA and PPB are plotted as shown in Fig.4.The red-shifts ͓͗E ͘(t )Ϫ͗E 0͔͘of about 140and 60meV are mea-sured within the ﬁrst 100ps for PPA and PPB,respectively.Figures 5͑a ͒and 5͑b ͒show the transient behaviors of the PL intensity at three spectral positions for PPA and PPB,respectively.The PL decay of PPA consists of two compo-nents,an initial ultrafast decay and a following slow relax-ation.By using a biexponential response function,the time constants of the initial ultrafast decay are ﬁt to 5,5.7,and 6.7ps for the photon energy of 2.7,2.5,and 2.3eV,respectively,which indicates the dependence of the initial PL decay on the photon energy.The following slow decay processes for the three photon energies ͑2.7,2.5,and 2.3eV ͒are almost the same of about 180ps.However,the PL decay of PPB exhib-its a single exponential process with time constants of 112,125,and 210ps for the photon energy of 2.8,2.7,and 2.5eV,respectively.The dependence of the decay times on pho-ton energy for PPB becomes signiﬁcant in the slow decay process.The difference of the PL behaviors observed in PPA and PPB can be explained by their different substitutes attached on the main backbones.With double substitutions of phenyl and butyl,PPB molecules possess longer and more bulky side chains than that of PPA molecules with single phenyl substituent.As a result,the interchain transport of the excited states to the longer-conjugation-length segments 9is facili-tated between PPA molecules.The interchain migration,oc-curring following photoexcitation and prior to emission,is proposed as a nonradiative process,which is extremely rapid and can reduce the luminescence effectively.4,10This results in an ultrafast initial relaxation and a large red shift of tran-sient PL spectrum for PPA.Similar results have been ob-served in other EL polymers.2However,the longerdistanceFIG.3.Time resolved photoluminescence spectra of ͑a ͒PPA and ͑b ͒PPB after excitation with 150fs laser pulses at 3.2eV.FIG.4.Energetic positions of the main emission bands in ͑a ͒PPA and ͑b ͒PPB vs delay time.between adjacent PPB molecule main chains hampers the interchain transport and conﬁnes the relaxation process on the intrachain,4,8which lead to the absence of an initial ul-trafast PL decay in PPB.The red-shift of transient PL spec-trum and the dependence of decay time on photon energyobserved in PPB are probably due to chain distortion towards a more planar conformation following photoexcitation.3The bulky side groups attached on PPB molecular main chains may have a tendency to decrease the torsion angle of the repeat unit surface on the main chain,4which therefore lead to a small red-shift of the spectrum and slow PL decay.Con-sequently higher PL efﬁciency was observed for PPB.In conclusion,femtosecond PL studies of PPA and PPB demonstrate that side groups of substituted polyactylene molecule play an important role in the transient PL behavior.PPB molecules have longer and more bulky side groups than PPA molecule.As a result,PPB exhibits a much slower PL decay and a higher PL efﬁciency.Since EL and PL observed in conjugated polymers originate from the same recombina-tion processes,2,4we believe that our results are of particular interest for the design of new EL devices.The authors gratefully acknowledge technical assistance during the experiments by Dr.H.Wang and providing PPA and PPB samples by Dr.B.Z.Tang.They also thank Pro-fessor W.Z.Lin for careful reading of the manuscript and helpful suggestions.1R.Kersting,V.Lemmer,R.F.Mahrt,K.Leo,H.Kurz,H.Bassler,and E.O.Gobel,Phys.Rev.Lett.70,3820͑1993͒.2U.Lemmer,R.F.Mahrt,Y.Wada,A.Greiner,H.Bassler,and E.O.Gobel,Appl.Phys.Lett.62,2827͑1993͒.3uchlan,S.Etemad,T.C.Chung,A.J.Heeger,and A.G.Macdi-armid,Phys.Rev.B 24,3701͑1981͒.4R.Sun,T.Masuda,and T.Kobayashi,Jpn.J.Appl.Phys.,Part 235,L1434͑1996͒.5P.W.Hong,Thesis,Hong Kong University of Science and Technology,Thesis CHEM,C.2͑1996͒.6B.Z.Tang,W.H.Poon,S.M.Leung,W.H.Leung,and H.Peng,Mac-romolecules 38,1573͑1996͒.7J.Shah,T.C.Damen,B.Deveud,and Dominigue Block,Appl.Phys.Lett.50,1307͑1987͒.8R.Sun,T.Masuda,and T.Kobayashi,Jpn.J.Appl.Phys.,Part 235,L1673͑1996͒.9H.S.Woo,S.C.Graham,D.A.Halliday,D.D.C.Bradley,R.H.Friend,P.L.Burn,and A.B.Holmes,Phys.Rev.B 46,7379͑1992͒.10R.H.Friend,D.D.C.Bradley,and P.D.Townsend,J.Phys.D 20,1376͑1987͒.FIG.5.Time resolved photoluminescence decay of ͑a ͒PPA and ͑b ͒PPB,detected at different luminescence energies.。