THUSSAT中学生标准学术能力测试诊断性测试2020年5月测试测试清华大学文科综合一卷试题历史答案
【5月清华THUSSAT调考英语】2020年清华THUSSAT高三毕业班诊断性测试英语试卷(一卷)含答案解析

中学生标准学术能力诊断性测试2020年5月测试英语试卷本试卷共150分,考试时间100分钟。
第一部分阅读理解(共两节,满分60分)第一节(共15小题;每小题3分,满分45分)ASummer is that time of this year 2019 when everyone looks to escape from the boring activities of life. So bring your kids to join us to have fun in the summer camp.Outdoor Word Game:All you need to do is setting up many huge letters that can be easily moved. These letters will help kids spell out certain words. Teenagers would also love something like this as it gives them the opportunity to invest their time in learning new words.Spray Painting:To bring out the creativity in a person, an activity centered on spray painting is the perfect way to get those creative juices flowing. All you need to do is providing plain white T-shirts and some cans of colored spray paint. It will be quite surprising to see some of the original ideas that will flow from individuals.Outdoor Twister:A person will be in charge of monitoring the game and will call out colors which can be placed outdoors. Participants have the task of placing either their hands or legs on the same color. A fun and exciting game focuses on hand-eye coordination(协调).The Maze(迷宫):One of the funniest games is the hallway maze game where a maze is constructed from either toilet paper or strings of paper. The chosen participant is then asked to make his way through the maze within a limited time without touching paper. This is one of the best and entertaining summer camp ideas for kids and adults also.1.Which activity most probably needs some imagination?A.Outdoor Word Game. B.Spray Painting.C.Outdoor Twister. D.The Maze.2.Which of the following is TRUE according to the passage?A.The maze is made of toilet paper in The Maze.B.You have to spray juice on your T-shirt in Spray Painting.C.Outdoors Twister tests whether you are good at controlling your body movement.D.You have to bring some huge letters to play in Outdoor Word Games.3.Who is the article intended for?A.Parents. B.Children. C.Teachers. D.Teenagers.BWhat makes a person sexy? Looks, talent, public image and how we accordingly view their personality all play into why we find ourselves attracted to certain celebrities and public figures.Every year, People put it all together when they name their “Sexiest Man Alive”. This year, the magazine decided that United States musician John Roger Stephens, better known as John Legend, has the full package.The title comes following some impressive achievements for the 40-year-old. He is among the youngest people to win an Emmy, Grammy, Oscar and Tony award, and the first African-American man to win all four of them. With numerous awards and nominations, an Ivy-League degree, and a handsome face, Legend has gained huge popularity worldwide. However, his dedication to family life maybe what wins over many.After getting married to US model Chrissy Teigen in 2013, Legend is the father of one son and one daughter. He’s not shy about telling the world their love is permanent. On the inside of Legend’s arm, there is a tattoo that says “Chrissy Luna Miles”, the names of his wife and children. In fact, Legend has gained strength from his family since childhood. He still remembers the days when his family would gather around his grandmother’s piano to sing Christmas songs. This inspired his passion for music. His best-known single, All of Me, is a love song to his wife. When he performed it at the 2014 Grammy Awards, many were touched by his delicate voice and the deep love beneath. “The more you know someone, the more you’re inspired by your relationship,” Legend told LA Confidential Magazine. “You have to grow enough to be able to write that song with sincerity and authenticity.”Despite his onstage confidence, Legend had to work to become a legend. As a teenager, he was the smallest kid in class. “I was always less mature, less at ease socially. I had plenty of awkward times,” he told People. “Most of your teenage years, into your twenties, you’re still figuring out who you are, how you present yourself to the world ... Eventually, I think you get more at ease with yourself.” Indeed, knowing who you are and be yourself could be the real key to attractiveness.4.What plays the biggest part in making Legend popular worldwide?A.High-level education. B.Handsome look.C.Efforts put in family. D.Talent in music.5.What does the third paragraph mainly talk about?A.L egend’s hobby as a boy.B.L egend’s family members.C.The secret behind Legend’s success.D.Composing background of the song All of Me.6.What can you infer from the passage?A.Legend is proud of his success in music.B.Legend’s interest in music originates from his family.C.Legend is the first black singer who won the Grammy award.D.Legend received the title “Sexiest Man Alive” before he got married.7.What is essential to attractiveness according to Legend?A.Being more mature.B.Being true to yourself.C.Having onstage confidence.D.Having attractive appearance.CWhat makes people trustworthy? Do they always tell the truth? Do they always keep their promises? In the near future, voluntary blood donations might be used as a clue to prove someone’s trustworthiness.On Nov 19, the National Health Commission (NHC) and other departments jointly released a document urging the social credit system to include voluntary blood donations. An incentive mechanism(激励机制)is also recommended, to provide donors with more conveniences and rewards like preferential treatment in using public facilities or visiting government-run p arks, China Daily reported. “We need to show we care about donors so they feel honored,” Liu Jiang, director of Beijing’s blood donation office, told CCTV.Indeed, donating blood is key to saving many lives. Due to blood’s very limited shelf life, regular blood donations are needed from a sufficient number of healthy people. This ensures that safe blood will be available whenever and wherever it is needed, according to the World Health Organization. However, the notice received mixed reviews on social media, with some people questioning whether it was necessary to include voluntary blood donation in the social credit system, according to the South China Morning Post. The system records things like a person’s career information, and payments for credit cards or other debt expenses are used to tell whether a person or company is trustworthy. People who cannot pay debts on time would have a worse credit score, resulting in more difficulties borrowing money from banks, reported the People’s Daily.Many Sina Weibo users believe that blood donations should be voluntary. They say it has little to do with social credit. Others doubt whether it is appropriate to reward this action because some people would donate blood for reasons other than helping save people’s lives. Guangming Daily commented that only acts closely linked to personal credit should be included in the social credit system. A voluntary blood donor might refuse to pay his or her debts, while someone who doesn’t donate blood might have a perfect credit re cord, it noted.8.What benefit can voluntary blood donors get according to the 2nd paragraph?A.Free park tour. B.Free treatment in hospitals.C.Running in government parks. D.Priority in using public facilities.9.Why are blood donations regularly needed?A.Blood can’t be stored for long.B.Some donated blood is not healthy.C.Fewer people are willing to donate blood.D.More and more people need blood to get treated.10.What can you learn from the last paragraph?A.Many blood donors refuse to pay his or her debts.B.Whoever donates blood will have a perfect credit record.C.It’s right to reward blood donators for they save people’s lives.D.Some people agree that voluntary blood donation shouldn’t be linked to social credit. 11.What’s the author’s attitude to using voluntary blood donations to prove trustworthiness?A.Positive. B.Objective. C.Indifferent D.Negative.DFive years ago, UK schools began to learn Shanghai’s math teaching methods on account of top scores produced by Chinese teenagers in a global academic evaluation. This test is called the Program for International Student Assessment (PISA), run by the organization for Economic Cooperation and Development (OECD). Taking around three hours on computers, it mainly focuses on 15-year-olds’ reading, math and science abilities. Earlier this month, the latest test results were published.Students from Beijing and Shanghai, as well as Jiangsu and Zhejiang provinces, outperformed students from the other 78 participating education systems in mathematics and science by a wide margin. In reading comprehension scores, only Singapore came close. Chinese mainland students first took the tests in 2009, with Shanghai topping the scores. The city claimed the top spot again in 2012.The PISA rankings aroused global interest in Chinese teaching methods and as a result, Britain increased exchanges with Chinese schools to improve its math education. Nearly 600 teachers from Britain and China, mainly from primary schools, have taken part in the ex change since 2014, and around 5,000 of Britain’s 16,000 primary schools have adopted the Shanghai math teaching method. In the latest PISA test, Chinese mainland students averaged 591 points in math —more than 100 points higher than the OECD average. British students averaged 502, jumping from 27th place three years ago to 18th in the math rankings.Philip Avery, director of education for the Bohunt Education Trust, UK, said, “We’ve benefited fromworking with Chinese partners for the high quality of lesson planning and teaching for memorization in China. Our Chinese partners have benefited from our approach to the development of character, focus on innovation and application of knowledge.”However, Zhang Xuanmin, who is in charge of the PISA project in Shanghai, points out that besides basic education, Chinese students still need to improve their social and emotional competencies. 12.Which of the following is TRUE according to the passage?A.UK teenagers are poorly-educated.B.UK schools’ teaching methods are out of fashion.C.Teenagers in Shanghai got higher scores in PISA.D.Teenagers in Shanghai are the best students in the world.13.What does the underlined word mean in the 2nd paragraph?A.Laugh at. B.Look up to. C.get along with. D.be superior to. 14.What’s probably the average math point of OECD in the latest PISA test?A.302. B.490. C.502. D.591.15.What can be indicated by Philip Avery’s words?A.The exchange program is a win-win situation.B.Chinese students still have a long way to go in education.C.UK schools get more benefits from the exchange program.D.UK teenagers have gained a lot of improvement in math learning.第二节(共5小题;每小题3分,满分15分)根据短文内容,从短文后的选项中选出能填入空白处的最佳选项。
清华大学中学生标准学术能力诊断性测试2020届高三5月测试理综试题 Word版含答案

中学生标准学术能力诊断性测试2020年5月测试理科综合试卷(一卷)本试卷共300分,考试时间150分钟。
可能用到的相对原子质量:H 1 O 16 C 12 N 14 Fe 56 Cr 52 S 32 I 127 Ba 137一、选择题:本题共13小题,每小题6分,共78分。
在每小题给出的四个选项中,只有一项是符合题目要求的。
1.2019年诺贝尔生理医学奖授予了揭示“细胞如何感知和适应氧气”机理的三位科学家。
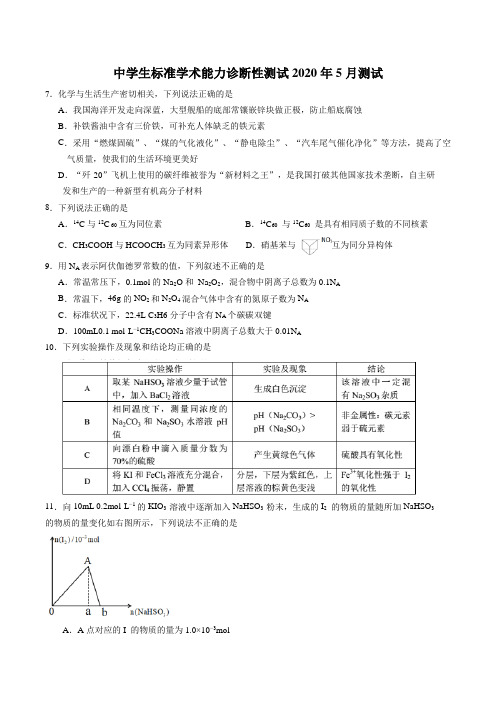
他们研究发现肾脏中有专门的细胞可以感受氧气水平的变化,当氧气供应不充足时,低氧诱导因子(HIF-1α)不被降解,细胞内积累的HIF-1α可促进促红细胞生成素(EPO)的合成;氧气供应充足时,HIF-1α合成后很快被降解,具体调节途径如下图所示。
请据图分析下列说法错误的是A.低氧时,HIF-1α进入细胞核联合ARNT与EPO基因调控序列结合,增强EPO基因的表达,使细胞合成更多的EPOB.若将细胞中的脯氨酸酰羟化酶基因敲除,EPO基因的表达水平会下降,细胞合成的EPO量减少C.正常条件下,人体细胞内HIF-1α的含量较低,在医学上可尝试通过提高HIF-1α的含量来治疗人类的贫血症D.高原反应时,EPO 会刺激骨髓中的造血干细胞,使其增殖分化生成大量红细胞,提高氧气的运输能力,该调节方式属于激素调节2.双脱氧核苷三磷酸(ddNTP)属于非天然核苷酸,是桑格-库森法测定DNA 序列的底物。
ddNTP 与脱氧核苷三磷酸(dNTP)的结构如下图所示,根据所学知识判断下列选项错误的是A.若X表示OH,该结构代表的物质dNTP可作为体内DNA分子复制的原料B.若将ddNTP加到正在复制的DNA反应体系中,ddNTP结合到子链后,子链的延伸会立即终止C.ddNTP、dNTP以及磷脂分子的元素组成都为C、H、O、N、PD.若X表示OH,碱基为A时,该物质可为细胞的各项生命活动直接提供能量3.细胞自噬有助于人类更好地了解细胞如何实现自身的循环利用,帮助细胞适应饥饿和应对感染等不良环境。
清华大学中学生标准学术能力诊断性测试2020届高三5月测试数学(文)试题(一卷)与答案

中学生标准学术能力诊断性测试2020年5月测试文科数学试卷(一卷)本试卷共150分,考试时间120分钟。
一、选择题:本大题共12小题,每小题5分,共60分.在每小题给出的四个选项中,只有一项是符合题目要求的.1.已知集合{}14P x x =-<<,{}2Q x x =<,则()R P Q ⋂=ð( ) A .[)2,4 B .()1,-+∞ C .[)2,+∞ D .(]1,2- 2.已知复数z 满足4zi i=-(其中i 为虚数单位),则z 的虚部为( ) A .4iB .4C .1D .1-3.已知实数x ,y 满足约束条件222y x y x ≤⎧⎪+≥⎨⎪≤⎩,则3x y +的最大值为( )A .2B .6C .8D .124.已知实数a ,b 满足5a b +=,23log log a b =,则ab =( ) A .2B .3C .5D .65.已知向量a r ,b r 满足1a =r ,2a b -=r r且a b ⊥r r ,则向量a r 与a b -r r 的夹角为( )A .2π3B .π3C .5π6D .π66.已知函数()ln f x x x =,则函数()f x 的单调递增区间为( ) A .RB .()0,+∞C .1,e ⎛⎫+∞ ⎪⎝⎭D .(),e +∞7.数列{}n a 的前n 项和()2*23n S n n n =-∈N ,若5p q +=()*,p q ∈N ,则p q a a +=( ) A .6B .8C .9D .108.已知x ,y ∈R ,“1x y +≤且1x y -≤”是“1x y +≤”的( ) A .充分不必要条件B .必要不充分条件C .充要条件D .既不充分也不必要条件9.已知实数0a ≠,则函数()1sin f x ax a ⎛⎫=+⎪⎝⎭的图象一定不可能的是( )A .B .C .D .10.已知x ,y ∈R ,则方程组21y =⎪=+⎩的解(),x y 的个数( )A .0B .1C .2D .411.已知ABC △中,角A ,B ,C 所对的边分别是a ,b ,c .若4cos a b C b a +=,且()1cos 6A B -=,则cos C =( ) A .23B .34C .23或34- D .不存在12.已知m ,n ,p ∈R ,若三次函数()32f x x mx nx p =+++有三个零点a ,b ,c ,且满足()()3112f f -=<,()()022f f =>,则111a b c++的取值范围是( ) A .1,13⎛⎫⎪⎝⎭B .11,43⎛⎫⎪⎝⎭C .11,42⎛⎫⎪⎝⎭D .11,32⎛⎫⎪⎝⎭二、填空题:13.已知1F 和2F 是椭圆2213x y +=的两个焦点,则12F F =______. 14.将1名同学和2名老师随机地排成一排,则该名学生恰好在2名老师中间的概率为______. 15.定义在R 上的偶函数()f x 满足()184f x +=,则()2020f =______.16.已知ABC △中,π2A ∠=,3AB =,4AC =.如图,点D 为斜边BC 上一个动点,将ABD △沿AD 翻折,使得平面AB D '⊥平面ACD .当BD =______时,B C '取到最小值.三、解答题:解答应写出文字说明、证明过程或演算步骤.第17~21题为必考题,每道试题考生都必须作答.第22、23题为选考题,考生根据要求作答. (一)必考题:17.如图,点0P 是锐角α的终边与单位圆的交点,0OP 逆时针旋转π3得1OP ,1OP 逆时针旋转π3得2OP ,…,1n OP -逆时针旋转π3得n OP .(1)若0P 的坐标为34,55⎛⎫⎪⎝⎭,求点1P 的横坐标; (2)若点2020P 的横坐标为45,求2πsin 23α⎛⎫+ ⎪⎝⎭的值.18.某高中某班共有40个学生,将学生的身高分成4组:平频率/组距[)150,160,[)160,170,[)170,180,[)180,190进行统计,作成如图所示的频率分布直方图.(1)求频率分布直方图中a 的值和身高在[)160,170内的人数;(2)求这40个学生平均身高的估计值(同一组中的数据用该组区间的中点值为代表)(精确到0.01).19.如图,四棱锥P ABCD -中,PAB △是边长为2的等边三角形.梯形ABCD 满足:1BC CD ==,AB CD ∥,AB BC ⊥.(1)求证:PD AB ⊥;(2)若2PD =,求点D 到平面PBC 的距离.20.如图,已知抛物线C :24x y =,过直线1y =上一点M 作直线l 交抛物线C 于A ,B 两点,且点M 为AB 中点、作直线MN AB ⊥交y 轴于点N .(1)求点N 的坐标;(2)求NAB △面积的最大值. 21.已知函数()()1ln f x x x =+. (1)求()y f x =在1x =处的切线方程:(2)已知实数2k >时,求证:函数()y f x =的图象与直线l :()1y k x =-有3个交点.(二)选考题:共10分.请考生在第22,23题中任选一题作答,如果多做,则按所做的第一题计分.作答时请写清题号.22.[选修4-4:坐标系与参数方程选讲] 在极坐标系中,已知曲线C :2221sin ρθ=+,过点()1,0F -引倾斜角为α的直线l ,交曲线C 于P ,Q 两点.(1)求曲线C 的直角坐标方程;(2)若直线l 分别交直线2x =±于A ,B 两点,且PQ 、AF BF -、AB 成等比数列,求cos α的值. 23.[选修4-5:不等式选讲] 已知实数a ,b 满足:22a b +=. (1)求证:2821ca b b c +++≥+;(2)若对任意的a ,*b ∈R ,1211c c b a++-≤+恒成立,求c 的取值范围.中学生标准学术能力测试诊断性测试2020年5月测试文科数学(一卷)答案一.选择题:本大题共12小题,每小题5分.在每小题给出的四个选项中,只有一项是符合题目要求的.二.填空题:本大题共4小题,每小题5分.13.14.1315.38+ 16.157三、解答题:解答应写出文字说明、证明过程或演算步骤.第17~21题为必考题,每个试题考生都必须作答.第22、23题为选考题,考生根据要求作答. (一)必考题 17.解:(1)因为点034,55P ⎛⎫ ⎪⎝⎭,根据三角函数的定义可得4sin 5α=,3cos 5α= 根据题意可知点1P 的横坐标为πππ314cos cos cos sin sin 333525ααα⎛⎫+=-=⨯-=⎪⎝⎭ (2)根据题意可知点2020P 的横坐标为2020π4π4cos cos 335αα⎛⎫⎛⎫+=+= ⎪ ⎪⎝⎭⎝⎭ 所以π4cos 35α⎛⎫+=- ⎪⎝⎭又因为π0,2α⎛⎫∈ ⎪⎝⎭,所以ππ5π,336α⎛⎫+∈ ⎪⎝⎭,所以π3sin 35α⎛⎫+= ⎪⎝⎭所以2πππ24sin 22sin cos 33325ααα⎛⎫⎛⎫⎛⎫+=++=- ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭. 18.解:(1)由图可得[)150,160,[)160,170,[)170,180,[)180,190三组的频率分别为0.1250,0.3000,0.1250,可得人数分别为5,12,5, 所以身高在[)160,170内的共有18人, 所以180.04504010a ==⨯;(2)这40个学生平均身高的估计值为()1155516518175121855169.2540⨯⨯+⨯+⨯+⨯= 所以这40个学生平均身高的估计值为169.25cm . 19.解(1)取AB 的中点O ,连接OP ,OD ,因为PAB △为边长为2的等边三角形, 所以PO AB ⊥,因为1BO CD ==,AB CD ∥, 所以四边形OBCD 为平行四边形, 又因为AB BC ⊥,所以DO AB ⊥. 因为DO PO O ⋂=,所以AB ⊥平面POD , 所以PD AB ⊥;(2)设点D 到平面PBC 的距离为h ,因为1BC DO ==,PO =,2PD =,所以DO PO ⊥,又因为DO AB ⊥,所以DO ⊥平面PAB . 由D PBC P DBC V V --=可得,11113232h BC PB PO BC DC ⨯⨯⨯⨯=⨯⨯⨯⨯,所以2h =. 20.解:设点()11,A x y ,()22,B x y ,中点(),1M t ,直线AB 的斜率为k ,(k 斜率显然存在且不为0).由21122244x y x y ⎧=⎨=⎩可得()()()1212124x x x x y y -+=-, 所以1212124y y x x x x -+=⨯-,故24t k =,(1)直线MN :()11y x t k -=--,即()112y x k k-=--,解得点()0,3N . (2)因为直线AB 经过点(),1M t ,直线AB 的斜率为k , 所以可得直线AB 的方程是:221y kx k =-+,由22421x y y kx k ⎧=⎨=-+⎩联立可得224840x kx k -+-=, 所以1221224,84,16160,x x k x x k k +=⎧⎪=-⎨⎪∆=-+>⎩,所以12AB x =-=|2k2+2|又因为点N 到直线AB的距离为d =,所以NAB △的面积21S=+=≤=当213k=时,NAB△的面积取到最大值9.21.解:(1)因为()()1lnf x x x=+,所以()1lnxf x xx+'=+,所以()12f'=,又因为()10f=,所以()f x在1x=处的切线方程22y x=-;(2)证明:当2k>时,函数()y f x=的图象与直线l交点的个数等价于函数()()1ln1k xh x xx-=-+的零点个数,因为()()()()222121211x kxkh xx x x x+-'=-=++,()0,x∈+∞,设()()2221g x x k x=+-+,因为二次函数()g x在x∈R时,()010g=>,()1420g k=-<,所以存在()10,1x∈,()21,x∈+∞,使得()10g x=,()20g x=,所以()h x在()10,x单调递增,()12,x x单调递减,()2,x+∞单调递增.因为()10h=,所以()()110h x h>=,()()210h x h<=,因此()h x在()12,x x存在一个零点1x=;又因为当kx e-=,()()()1211k kkk kk e k eh e ke e-------=--=<++,所以()h x在()1,k e x-存在一个零点;当kx e=时,()()1211kkk kk eh e k ke e-⎛⎫=-=>⎪++⎝⎭,所以()h x在()2,kx e存在一个零点;所以,函数()y f x=的图象与直线l:()1y k x=-有3个交点.(二)选考题:请考生在第22,23题中任选一题作答,如果多做,则按所做的第一题计分.作答时请写清题号.22.[选修4-4:坐标系与参数方程]解:(1)曲线C 的方程可化为2212x y +=; (2)设直线l 的参数方程为1cos ,sin x t y t αα=-+⎧⎨=⎩(t 为参数),代入椭圆C 方程得()221sin 2cos 10t t αα+--=,所以1222cos 1sin t t αα+=+,12211sin t t α-=+,故12PQ t t =-=, 又因为4cos AB α=,2=cos AF BF α-,所以244cos cos αα= 当cos 0α≥时,2cos 20αα+-=,解得cos 2α=, 当cos 0α<时,2cos20αα--=,解得cos 2α=,所以cos 2α=2.∴曲线2C的直角坐标方程为20x -+=或100x -=.23.[选修4-5:不等式选讲](1)解:(1)证明:因为2224a b b a b +++≥++=, 若0c ≤,不等式显然成立;. 若0c >,则288411c c c c=≤=++, 所以2821ca b b c +++≥+, 当()()20a b b +⨯+≥,且1c =取到等号; 综上2821ca b b c +++≥+.(2)因为122222422a b a b a b b a b a b a+++=+=++≥, 所以114c c ++-≤,解得22c -≤≤.。
2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(文)试题(一卷)(解析版)

2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(文)试题(一卷)一、单选题1.已知集合{}{}14,2P x x Q x x =-<<=<,那么()R P C Q ⋂=( ) A .[)2,4B .()1,-+∞C .[)2,+∞D .(]1,2- 【答案】A 【解析】{}2R C Q x x =≥,所以()[)2,4R P C Q ⋂=,选A.2.已知复数z 满足4z i i =-(其中i 为虚数单位),则z 的虚部为( ) A .4iB .4C .1D .1- 【答案】B【解析】把已知等式变形,再由复数代数形式的乘除运算化简得答案.【详解】 由4z i i=-,得2(4)414z i i i i i =-=-=+. ∴复数z 的虚部是4.故选:B .【点睛】本题考查复数代数形式的乘除运算,考查复数的基本概念,是基础题.3.已知实数x ,y 满足约束条件222y x y x ≤⎧⎪+≥⎨⎪≤⎩,则3x y +的最大值为( )A .2B .6C .8D .12 【答案】C【解析】先根据约束条件画出可行域,再利用几何意义求最值,3z x y =+表示直线在y 轴上的截距,只需求出可行域直线在y 轴上的截距最大值即可.【详解】先根据约束条件画出可行域,作直线30x y +=,讲直线30x y +=平移,当过点(2,2)时,3x y +取得最大值 ()max 33228x y +=⨯+=故选:C .【点睛】本题主要考查线性规划问题,以及利用几何意义求最值,属于基础题.4.已知实数a ,b 满足5a b +=,23log log a b =,则ab =( )A .2B .3C .5D .6【答案】D【解析】设23log log a b k ==,则2k a =,3k b =,再利用5a b +=即可求出k 的值,进而求出a ,b 的值.【详解】设23log log a b k ==,则2k a =,3k b =,235k k a b ∴+=+=, 1k ∴=,2a ∴=,3b =,∴236ab =⨯=故选:D .【点睛】本题主要考查了对数式与指数式的互化,以及对数的运算性质,是基础题.5.已知向量a r ,b r 满足1a =r ,2a b -=r r 且a b ⊥r r ,则向量a r 与a b -r r 的夹角为( )A .2π3B .π3C .5π6D .π6【答案】B【解析】根据()a b b -⊥r r r 即可得出()0a b b -=r r r g ,从而得出·1a b =r r ,进而得出1cos ,2a b <>=r r ,根据向量夹角的范围即可求出夹角. 【详解】Q a b ⊥r r ,∴0a b ⋅=r r∴()21a a b a a b ⋅-=-⋅=r r r r r r 设向量a r 与a b -r r 的夹角为θ∴()1cos 2a a b a a b θ⋅-==-r r r r r r ∵()0,θπ∈∴3πθ=故选:B .【点睛】考查向量垂直的充要条件,以及向量数量积的运算,向量夹角的余弦公式,属于中档题. 6.已知函数()ln f x x x =,则函数()f x 的单调递增区间为( )A .RB .()0,∞+C .1,e ⎛⎫+∞ ⎪⎝⎭D .(),e +∞【答案】C【解析】求()ln f x x x =的导数()f x ',由()0f x '>,即可求得答案.【详解】()ln 1f x x '=+Q , 令()0f x '>得:ln 1x >-,11x e e-∴>=. ∴函数()ln f x x x =的单调递增区间为1,e ⎛⎫+∞ ⎪⎝⎭. 故选:C .【点睛】本题考查利用导数研究函数的单调性,易错点在于忽视函数的定义域,属于中档题.7.数列{}n a 的前n 项和()2*23n S n n n =-∈N ,若5p q +=()*,p q ∈N ,则p q a a +=( )A .6B .8C .9D .10【答案】D 【解析】当1n =时,可得1a ,当2n …时,1n n n a S S -=-,验证1n =时是否适合可得通项公式,代入通项公式求解可得结果.【详解】当1n =时,11231a S ==-=-,当2n …时,221232(1)3(1)45n n n a S S n n n n n -=-=---+-=-, 当1n =时,上式也适合,∴数列{}n a 的通项公式为:45n a n =-∴()454541010p q a a p q p q +=-+-=+-=故选:D .【点睛】本题考查等差数列的前n 项和公式和通项公式的关系,属中档题.8.已知x ,y ∈R ,“1x y +≤且1x y -≤”是“1x y +≤”的( )A .充分不必要条件B .必要不充分条件C .充要条件D .既不充分也不必要条件【答案】C【解析】作出不等式组对应的平面区域,利用区域关系结合充分条件和必要条件的定义进行判断即可.【详解】 1x y +≤且1x y -≤等价于1111x y x y -≤+≤⎧⎨-≤-≤⎩ 1x y +≤等价于()()()()10,010,010,010,0x y x y x y x y x y x y x y x y ⎧+≤≥≥⎪-≤≥≤⎪⎨-+≤≤≥⎪⎪--≤≤≤⎩作出两个不等式组对应的平面区域都是以()1,0,()0,1,()1,0-,()0,1-为顶点的正方形∴“1x y +≤且1x y -≤”是“1x y +≤”的充要条件,故选:C .【点睛】本题主要考查充分条件和必要条件的判断,结合充分条件和必要条件结合平面区域的关系是解决本题的关键.9.已知实数0a ≠,则函数()1sin f x ax a ⎛⎫=+ ⎪⎝⎭的图象一定不可能的是( ) A .B .C .D .【答案】A【解析】求函数的导数,判断()0f '的正负情况,即可得出答案.【详解】∵()1sin f x ax a ⎛⎫=+ ⎪⎝⎭ ∴()1cos f x a ax a ⎛⎫'=+⎪⎝⎭ ∴()10cos f a a'=, 观察各选项的图象,判断()0f '的正负情况,得:观察A 选项的图象,得()10sin 0f a=>,34T <<,故234a π<< ∴223a ππ<<,3122a ππ<<,()10cos 0f a a'=> 故A 选项的图象不符合观察B 选项的图象,得()10sin 0f a=>,67T <<,故267a π<< ∴273a ππ<<,3172a ππ<<,()10cos 0f a a'=> 故B 选项的图象符合观察C 选项的图象,得()10sin 0f a=>,69T <<,故269a π<< ∴293a ππ<<,3192a ππ<<,()10cos 0f a a'=> 故C 选项的图象符合观察D 选项的图象,得()10sin 0f a=>,24T <<,故224a π<< ∴2a ππ<<,112a ππ<<,()10cos 0f a a'=> 故D 选项的图象符合故选:A.【点睛】本题考查了函数的图象的识别,利用导数判断函数的性质,属于中档题.10.已知x ,y ∈R ,则方程组21y =⎪=+⎩的解(),x y 的个数( )A .0B .1C .2D .4【解析】2=的几何意义知图像是双曲线,化简得2213y x -=,故题目等价于求解直线1y =+与双曲线2213y x -=的交点个数,联立方程组求解即可.【详解】设()12,0F -,()22,0F ,(),P x y2=等价于212PF PF -=∴动点P 的轨迹是以()12,0F -,()22,0F 为焦点,以2为实轴长的双曲线∴2c =,1a =,b =∴双曲线的标准方差为2213y x -= ∴题目等价于求解直线1y =+与双曲线2213y x -=的交点个数 联立22131y x y⎧-=⎪⎨⎪=+⎩,求解得1x y ⎧=⎪⎨⎪=-⎩∵方程组只有一组解,故直线1y =+与双曲线2213y x -=只有一个交点 故选:B.【点睛】本题主要考查双曲线和直线的位置关系,根据定义判断得到曲线为双曲线是解题的关键.11.已知ABC V 中,角A ,B ,C 所对的边分别是a ,b ,c .若4cos a b C b a+=,且()1cos 6A B -=,则cos C =( ) A .23 B .34 C .23或34- D .不存在【解析】由题意,利用余弦定理和正弦定理,化简求得222sin sin 2sin A B C +=,再利用降幂公式与和差化积,以及同角的三角函数关系,求得cos C 的值.【详解】ABC ∆中,4cos b a C a b+=,cos 0C ∴>;2222222cos 2()a b ab c a b c ∴+=⨯=+-, 2222a b c ∴+=,222sin sin 2sin A B C ∴+=, ∴21cos21cos22sin 22A B C --+=, 即22(cos2cos2)4sin A B C -+=;22cos[()()]cos[()()]4sin A B A B A B A B C -++--+--=222cos()cos()4sin A B A B C ∴-+-=, 又1cos()6A B -=,cos()cos A B C +=-, 2212cos 4sin 4(1cos )3C C C ∴+==⨯-, 化简得212cos cos 60C C +-=,解得2cos 3C =或3cos 4C =- ∵4cos 2a b C b a =+≥,1cos 2C ≥ ∴2cos 3C =. 故选:A .【点睛】本题考查了三角恒等变换应用问题,也考查了正弦、余弦定理的应用问题,是中档题.12.已知m ,n ,p ∈R ,若三次函数()32f x x mx nx p =+++有三个零点a ,b ,c ,且满足()()3112f f -=<,()()022f f =>,则111a b c ++的取值范围是( ) A .1,13⎛⎫⎪⎝⎭B .11,43⎛⎫ ⎪⎝⎭C .11,42⎛⎫ ⎪⎝⎭D .11,32⎛⎫ ⎪⎝⎭【答案】D【解析】根据条件建立方程求出m ,n 的值,然后回代,求出p 的范围,结合零点式求出a ,b ,c 的等式关系,结合不等式的性质进行求解即可.【详解】∵()()3112f f -=<,()()022f f => ∴11842m n p m n p p m n p -+-+=+++⎧⎨=+++⎩,即10240n m n +=⎧⎨++=⎩, 得321m n ⎧=-⎪⎨⎪=-⎩,代入得323()2f x x x x p =--+, ∵()312f -<,()02f > ∴3311222p p ⎧--++<⎪⎨⎪>⎩,解得23p <<,设三次函数的零点式为()()()()f x x a x b x c =---,比较系数得1ab bc ca ++=-,abc p =-, 故1111ab bc ca a b c abc p ++++==∈11,32⎛⎫ ⎪⎝⎭故选:D.【点睛】本题主要考查函数与方程的应用,利用条件求出参数m ,n ,利用函数零点式以及不等式的关系进行转化是解决本题的关键.二、填空题13.已知1F 和2F 是椭圆2213x y +=的两个焦点,则12F F =______.【答案】【解析】求出椭圆的a ,b,再由c 2c .【详解】 椭圆2213x y +=的a =1b =,∴c ==即有12||F F =故答案为:.【点睛】本题考查椭圆的方程,主要考查椭圆的焦距的求法,考查运算能力,属于基础题. 14.将1名同学和2名老师随机地排成一排,则该名学生恰好在2名老师中间的概率为______. 【答案】13【解析】用列举法计算总的排法和该名学生恰好在2名老师中间的排法,由概率公式可得.【详解】设学生用a 表示,老师用A 、B 表示1名同学和2名老师随机地排成一排,总的排法有:aAB ,aBA ,AaB ,ABa ,BaA ,BAa ,共6种其中该名学生恰好在2名老师中间的有AaB ,BaA 共2种所以该名学生恰好在2名老师中间的概率为2163P ==. 故答案为:13. 【点睛】本题主要考查古典概型的计算,属于基础题.15.定义在R 上的偶函数()f x 满足()184f x +=,则()2020f =______.【解析】将等式中的x 替换为x -,两式相减得()()88f x f x +=-+,结合()f x 是偶函数,得到函数()f x 的周期8T =,所以()()()202044f f f ==-,令4x =-代入求解即可.【详解】∵()()()2184f x f x f x +=+-……①将①中的x 替换为x -,得()()()2184f x f x f x -+=+---……②①-②得()()880f x f x +--+=又∵()f x 是偶函数,故()()f x f x -= ∴()()()888f x f x f x +=-+=- ∴()f x 是周期函数,16T =∴()()()()202012616444f f f f =⨯+==- ①式中令4x =-,得()()()2148444f f f -+=+---∴()()()214444f f f =+-,整理得()()()()()2232424410440144f f f f f ⎧⎪-+=⎪-≥⎨⎪⎪≥⎩解得()374f +=∴()()20204f f ==37+ 故答案为:378+. 【点睛】本题主要考查函数的奇偶性和周期性,属于中档题. 16.已知ABC V 中,π2A ∠=,3AB =,4AC =.如图,点D 为斜边BC 上一个动点,将ABD △沿AD 翻折,使得平面AB D '⊥平面ACD .当BD =______时,B C '取到最小值.【答案】157【解析】设BAD ∠=α,作BE AD ⊥或AD 的延长线于E 点,作CF AD ⊥或AD 的延长线于F 点,求出BE 、CF 、EF ,表示出2512sin 2B C α'=-,利用三角函数性质求最值,B C '取最小值时,4πα=,在BAD V 中利用正弦定理可求BD 的值. 【详解】设BAD ∠=α,0,2πα⎛⎫∈ ⎪⎝⎭,作BE AD ⊥或AD 的延长线于E 点,作CF AD ⊥或AD 的延长线于F 点,则ACF BAD α∠=∠=,3sin BE α=,3cos AE α=,4cos CF α=,4sin AF α=∴4sin 3cos EF AF AE αα=-=- ∴2222512sin 2B C BE CF EF α'=++=-∴当sin21α=,即4πα=时,min 13B C ' 在Rt ABC V 中,4sin 5ABC ∠=,3cos 5ABC ∠= 在BAD V 中,由正弦定理得sin sin BD ABBAD ADB=∠∠ 即()3sin sin BD ABC αα=∠+ ∴()3sin 3sin 15sin sin cos cos sin 7BD ABC ABC ABC ααααα===∠+∠+∠.故答案为:157. 【点睛】本题主要考查空间中的线段长计算,考查正弦定理得应用,考查学生的计算能力,属于难题.三、解答题17.如图,点0P 是锐角α的终边与单位圆的交点,0OP 逆时针旋转π3得1OP ,1OP 逆时针旋转π3得2OP ,…,1n OP -逆时针旋转π3得n OP .(1)若0P 的坐标为34,55⎛⎫⎪⎝⎭,求点1P 的横坐标; (2)若点2020P 的横坐标为45,求2πsin 23α⎛⎫+ ⎪⎝⎭的值.【答案】(1343-2)2425- 【解析】(1)由题意利用任意角的三角函数的定义,求得cos α、sin α的值,再利用两角和的余弦公式即可求解;(2)根据得2020P 的横坐标4cos(2020)35πα+⨯=的值,化简得π4cos 35α⎛⎫+=- ⎪⎝⎭,利用同角三角函数关系和二倍角公式可求2πsin 23α⎛⎫+ ⎪⎝⎭的值. 【详解】(1)因为点034,55P ⎛⎫ ⎪⎝⎭,根据三角函数的定义可得4sin 5α=,3cos 5α=根据题意可知点1P 的横坐标为πππ3143343cos cos cos sin sin 333525ααα-⎛⎫+=-=⨯-=⎪⎝⎭ (2)根据题意可知点2020P 的横坐标为2020π4π4cos cos 335αα⎛⎫⎛⎫+=+= ⎪ ⎪⎝⎭⎝⎭ 所以π4cos 35α⎛⎫+=- ⎪⎝⎭ 又因为π0,2α⎛⎫∈ ⎪⎝⎭,所以ππ5π,336α⎛⎫+∈ ⎪⎝⎭,所以π3sin 35α⎛⎫+= ⎪⎝⎭所以2πππ24sin 22sin cos 33325ααα⎛⎫⎛⎫⎛⎫+=++=- ⎪ ⎪ ⎪⎝⎭⎝⎭⎝⎭. 【点睛】本题主要考查任意角的三角函数的定义,二倍角公式、诱导公式、两角和的余弦公式,属于中档题.18.某高中某班共有40个学生,将学生的身高分成4组:平频率/组距[)150,160,[)160,170,[)170,180,[)180,190进行统计,作成如图所示的频率分布直方图.(1)求频率分布直方图中a 的值和身高在[)160,170内的人数;(2)求这40个学生平均身高的估计值(同一组中的数据用该组区间的中点值为代表)(精确到0.01).【答案】(1)0.0450;18人,(2)169.25cm .【解析】(1)根据频率分布直方图和频率的定义可得a 的值,计算身高在[)160,170内的频率,由此能估计身高在[)160,170内的人数;(2)同一组中的数据用该组区间的中点值为代表,直接计算可得平均身高的估计值. 【详解】(1)由图可得[)150,160,[)170,180,[)180,190三组的频率分别为0.1250,0.3000,0.1250 所以10.12500.30000.12500.045010a ---==所以身高在[)160,170内的人数为:400.0451018⨯⨯=(人) (2)这40个学生平均身高的估计值为()1155516518175121855169.2540⨯⨯+⨯+⨯+⨯= 所以这40个学生平均身高的估计值为169.25cm . 【点睛】本题考查了频率分布直方图的应用以及平均数的计算问题,属于基础题.19.如图,四棱锥P ABCD -中,PAB △是边长为2的等边三角形.梯形ABCD 满足:1BC CD ==,AB ∥CD ,AB BC ⊥.(1)求证:PD AB ⊥;(2)若2PD =,求点D 到平面PBC 的距离. 【答案】(1)见解析(2)3【解析】(1)证明AB ⊥平面POD ,由PD ⊂平面POD ,从而得到PD AB ⊥; (2)利用等体积法D PBC P DBC V V --=计算即可得结果. 【详解】(1)取AB 的中点O ,连接OP ,OD ,因为PAB △为边长为2的等边三角形, 所以PO AB ⊥,因为1BO CD ==,AB ∥CD , 所以四边形OBCD 为平行四边形, 又因为AB BC ⊥,所以⊥DO AB . 因为DO PO O =I ,所以AB ⊥平面POD , 所以PD AB ⊥;(2)设点D 到平面PBC 的距离为h ,因为1BC DO ==,3PO =2PD =,所以DO PO ⊥, 又因为⊥DO AB ,所以DO ⊥平面PAB . 由D PBC P DBC V V --=可得,11113232h BC PB PO BC DC ⨯⨯⨯⨯=⨯⨯⨯⨯, 所以32h =. 【点睛】本题主要考查线面垂直的判定定理和点到平面的距离计算,利用等体积法是解决点到平面的距离的关键.20.如图,已知抛物线C :24x y =,过直线1y =上一点M 作直线l 交抛物线C 于A ,B 两点,且点M 为AB 中点、作直线MN AB ⊥交y 轴于点N .(1)求点N 的坐标; (2)求NAB △面积的最大值. 【答案】(1)()0,3N (2)166【解析】(1)设点()11,A x y ,()22,B x y ,中点(),1M t ,直线AB 的斜率为k ,利用点差法得2t k =,写出直线MN 的方程可得N 的坐标;(2)设出直线AB 的方程,与抛物线方程联立,利用弦长公式得2121AB k x x =+-,利用点到直线的距离公式得点N 到直线AB 的距离,进而表示出NAB ∆的面积,利用基本不等式确定三角形面积的最大值. 【详解】设点()11,A x y ,()22,B x y ,中点(),1M t ,直线AB 的斜率为k ,(k 斜率显然存在且不为0).由21122244x y x y ⎧=⎨=⎩可得()()()1212124x x x x y y -+=-, 所以1212124y y x x x x -+=⨯-,故24t k =,(1)直线MN :()11y x t k -=--,即()112y x k k-=--,解得点()0,3N . (2)因为直线AB 经过点(),1M t ,直线AB 的斜率为k , 所以可得直线AB 的方程是:221y kx k =-+,由22421x yy kx k ⎧=⎨=-+⎩联立可得224840x kx k -+-=, 所以1221224,84,16160,x x k x x k k +=⎧⎪=-⎨⎪∆=-+>⎩,所以12AB x =-= 又因为点N 到直线AB的距离为d =,所以NAB △的面积为:2112S AB d ==+=≤=当213k =时,NAB △ 【点睛】本题考查抛物线的性质、直线与抛物线的位置关系的应用,注意韦达定理、弦长公式、不等式等知识的灵活运用,考查运用解析几何的方法解决数学问题的能力,属于中档题. 21.已知函数()()1ln f x x x =+. (1)求()y f x =在1x =处的切线方程:(2)已知实数2k >时,求证:函数()y f x =的图象与直线l :()1y k x =-有3个交点.【答案】(1)22y x =-(2)见解析【解析】(1)求出原函数的导函数,可得()12f '=,再求出切点为(1,0),利用直线方程的点斜式可得函数的图象在1x =处的切线方程;(2)函数()y f x =的图象与直线l 交点的个数等价于函数()()1ln 1k x h x x x -=-+的零点个数,通过导数判断函数的单调性,求函数的最值同0进行比较,得到结果. 【详解】(1)因为()()1ln f x x x =+,所以()1ln x f x x x+'=+, 所以()12f '=,又因为()10f =,所以()f x 在1x =处的切线方程22y x =-;(2)证明:当2k >时,函数()y f x =的图象与直线l 交点的个数等价于函数()()1ln 1k x h x x x -=-+的零点个数, 因为()()()()222121211x kx k h x x x x x +-'=-=++,()0,x ∈+∞, 设()()2221g x x k x =+-+,因为二次函数()g x 在x ∈R 时,()010g =>,()1420g k =-<, 所以存在()10,1x ∈,()21,x ∈+∞,使得()10g x =,()20g x =, 所以()h x 在()10,x 单调递增,()12,x x 单调递减,()2,x +∞单调递增. 因为()10h =,所以()()110h x h >=,()()210h x h <=, 因此()h x 在()12,x x 存在一个零点1x =; 又因为当k x e -=,()()()12011k k k kkk e k e h e k e e -------=--=<++,所以()h x 在()1,ke x -存在一个零点;当k x e =时,()()12011k k kk k e h e k k e e -⎛⎫=-=> ⎪++⎝⎭, 所以()h x 在()2,kx e存在一个零点;所以,函数()y f x =的图象与直线l :()1y k x =-有3个交点.【点睛】本题考查利用导数研究过曲线上某点处的切线方程,考查运用导数研究函数性质的方法,考查运算能力,考查函数与方程的数学思想方法和分析问题、解决问题的能力. 22.在极坐标系中,已知曲线C :2221sin ρθ=+,过点()1,0F -引倾斜角为α的直线l ,交曲线C 于P ,Q 两点. (1)求曲线C 的直角坐标方程;(2)若直线l 分别交直线2x =±于A ,B 两点,且PQ 、AF BF -、AB 成等比数列,求cos α的值.【答案】(1)2212x y +=;(2)cos 2α=2【解析】(1)曲线C 的极坐标方程转化为222sin 2ρρθ+=,由此能求出曲线C 的直角坐标方程;(2)写出直线l 的参数方程,将直线l 的参数方程代入曲线C 的直角坐标方程,得()222cos 2cos 10tt αα--⋅-=,由此韦达定理、等比数列的性质,结合已知条件能求出cos α的值. 【详解】(1)∵曲线C :2221sin ρθ=+∴222sin 2ρρθ+=∴曲线C 的直角坐标方程为2222x y y ++=,化简得2212x y +=(2)直线l 的参数方程为1cos sin x t y t αα=-+⎧⎨=⎩直线l 的参数方程代入曲线C 的直角坐标方程,整理得:()222cos 2cos 10tt αα--⋅-=设P ,Q 两点对应的参数分别为1t ,2t ,则1222cos 2cos t t αα+=-,12212cos t t α-=-∴1222cos PQ t t α=-==- 设直线l 交直线2x =-于A 点,直线l 交直线2x =于B 点,∴1cos AF α=,3cos BF α=,4cos AB AF BF α=+=(2πα≠) ∵PQ 、AF BF -、AB 成等比数列 ∴()2AF BFPQ AB -=⋅代入数据得:22134cos cos 2cos cos αααα⎛⎫-=⋅ ⎪ ⎪-⎝⎭解得:cos 2α=或cos 2α=.【点睛】本题考查直角坐标方程、极坐标方程、参数方程的互化等基础知识,考查等比数列的性质,考查题目转换能力和运算求解能力,是中档题. 23.已知实数a ,b 满足:22a b +=. (1)求证:2821ca b b c +++≥+; (2)若对任意的a ,*b ∈R ,1211c c b a++-≤+恒成立,求c 的取值范围. 【答案】(1)见解析(2)22c -≤≤【解析】(1)利用基本不等式和绝对值的三角不等式证明; (2)利用基本不等式求出12b a+的最小值,得出114c c ++-≤,再讨论c 的范围解出c . 【详解】(1)证明:因为2224a b b a b +++≥++=, 若0c ≤,不等式显然成立;.若0c >,则288411c c c c=≤=++, 所以2821ca b b c +++≥+,当()()20a b b +⨯+≥,且1c =取到等号; 综上2821ca b b c +++≥+. (2)因为122222422a b a b a b b a b a b a+++=+=++≥,第 21 页 共 21 页 所以114c c ++-≤,当1c ≤-时,()114c c -++-≤,解得2c ≥-,∴21c -≤≤-;当11c -<<时,1124c c ++-=≤,∴11c -<<;当1c ≥时,114c c ++-≤,解得2≤c ,∴12c ≤≤.综上,解得22c -≤≤.【点睛】本题考查 了不等式的证明,绝对值不等式的解法,绝对值的三角不等式和基本不等式的应用,属于中档题.。
清华大学中学生标准学术能力诊断性测试2020届高三5月测试数学(理)试题(一卷)与答案

中学生标准学术能力诊断性测试2020年5月测试理科数学试卷(一卷)本试卷共150分,考试时间120分钟。
一、选择题:本大题共12小题,在每小题给出的四个选项中,只有一项是符合题目要求的. 1.已知集合{}2log (2)0A x x =->,{}245,B y y x x x A ==-+∈,则A B ⋃=( ) A .[)3,∞B .[)2,∞C .()2,∞D .()3,∞2.在复平面内,复数231ii+-的虚部为( )A B C .D . 3.已知单位向量a ,b 满足22a b a b +=-,则()()3a b a b -⋅+=( ) A .1B .2C .3D .44.下列程序框图的算法思想源于我国古代数学名著《九章算术》中的“更相减损术”.执行该程序框图,若输入16a =,10b =,则程序中需要做减法的次数为( )A .6B .5C .4D .35.在()()252211x x x ++-的展开式中,4x 的系数为( )A .6-B .6C .10D .46.在三角形ABC 中,a ,b ,c 分别为角A ,B ,C 的对边,且满足22265b c a bc +=+,则sin 2B C +⎛⎫=⎪⎝⎭( )A .2B C .5D7.函数()32x e f x x -=-的部分图像大致是( )A .B .C .D .8.已知函数()31663x x f x x x e e -=--+-,若211011f f a a ⎛⎫⎛⎫+≤ ⎪ ⎪+-⎝⎭⎝⎭,则a 的取值范围为( ) A .(][),12,-∞-⋃+∞ B .()1,2- C .()()1,00,1-⋃ D .()[)1,1$2,-⋃+∞9.已知等差数列{}n a 满足:11a =,4164a a +=,则1912222a a a⨯⨯⋅⋅⋅⨯=( )A .382B .192C .162D .76210.已知椭圆()222210x y a b a b +=>>21y x =+相切,则a =( )A .2BCD .111.设()f x 为定义于[]1,1-上的偶函数,当[]0,1x ∈时,()12f x x =-,则方程()()22x f f x =的实数解的个数为( ) A .8B .6C .4D .212.已知当[]0,1x ∈时,不等式()()22cos 11sin 0x x x x θθ--+->恒成立,则θ的取值范围为( ) A .π5πππ1212k k θ+<<+(k 为任意整数) B .π5πππ66k k θ+<<+(k 为任意整数) C .π5π2π2π1212k k θ+<<+(k 为任意整数) D .π5π2π2π66k k θ+<<+(k 为任意整数) 二、填空题:本大题共4小题.13.设数列{}n a 满足14a =,210a =,215n n a a -=,3n ∀≥,则201920181ln ln 2a a -=______. 14.设实数x ,y 满足02203x y x y x -≥⎧⎪++≥⎨⎪≤⎩,则22x y +的最大值为______.15.假设抛一枚质地均匀的色子,若抛出的点数为1、2或3,我们称为“小”,否则,若抛出的点数为4、5或6,则称为“大”。
2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(理)试题(一卷)(解析版)

中学生标准学术能力诊断性测试2020年5月测试理科数学试卷(一卷)本试卷共150分,考试时间120分钟.一、选择题:本大题共12小题,在每小题给出的四个选项中,只有一项是符合题目要求的. 1.已知集合{}2log (2)0A x x =->,{}245,B y y xx x A ==-+∈,则A B =U ( )A.[)3,+∞B.[)2,+∞ C.()2,+∞D.()3,+∞【答案】C 【分析】先解对数不等式求出集合A ,再根据二次函数的单调性求出集合B ,然后根据并集的定义求解即可.【详解】解:∵2log (2)0x ->, ∴21x ->,即3x >, ∴()3,A =+∞,∴()2245212y x x x =-+=-+>, ∴()2,B =+∞,∴A B =U ()2,+∞,故选:C .【点睛】本题主要考查集合的并集运算,考查对数不等式的解法,考查二次函数的值域,属于基础题. 2.在复平面内,复数231ii+-的虚部为( )C. D. 【答案】B 【分析】根据复数代数形式的除法运算和复数的模先化简该复数,再根据虚部的定义得出结论.【详解】解:∵)()()231111122ii i i i i i ++===+---+,∴复数231i i +-的虚部为,故选:B .【点睛】本题主要考查复数代数形式的除法运算,考查复数的模和虚部的定义,属于基础题.3.已知单位向量a r ,br满足22a b a b +=-r r r r ,则()()3a b a b -⋅+=r rr r ( )A. 1B. 2C. 3D. 4【答案】B 【分析】由22a b a b +=-r r r r 可得0a b ⋅=r r ,再根据平面向量的数量积的定义即可求出答案. 【详解】解:∵22a b a b +=-r r r r ,∴()()2222a ba b+=-r r r r ,化简得0a b ⋅=r r ,∵1a =r,1=r b ,∴()()223322a b a b a b a b -⋅+=-+⋅=r r r r rr r r ,故选:B .【点睛】本题主要考查平面向量数量积的定义及其应用,属于基础题.4.下列程序框图的算法思想源于我国古代数学名著《九章算术》中的“更相减损术”.执行该程序框图,若输入16a =,10b =,则程序中需要做减法的次数为( )A. 6B. 5C. 4D. 3【答案】C 【分析】由循环结构的特点,先判断,再执行,分别计算出当前的,a b 的值,即可得到结论. 【详解】解:由16a =,10b =,满足a b ¹,满足a b >,则16106a =-=; 满足a b ¹,不满足a b >,则1064b =-=; 满足a b ¹,满足a b >,则642a =-=; 满足a b ¹,不满足a b >,则422b =-=;不满足a b ¹,则输出2a =; 则程序中需要做减法的次数为4, 故选:C .【点睛】本题主要考查算法和程序框图,主要考查循环结构的理解和运用,以及赋值语句的运用,属于基础题. 5.在()()252211x x x ++-的展开式中,4x 的系数为( )A. 6-B. 6C. 10D. 4【答案】A 【分析】 因为()()()()2545221111x x x x x ++-+-=,且()41+x 的展开式的通项公式为14r r r T C x +=,()51x -的展开式的通项公式为()5151kk k k T C x -+=⋅-⋅,令4r k +=,由此可求出答案.【详解】解:∵()()()()2545221111x x x x x ++-+-=,∵()41+x 的展开式的通项公式为41441rrr r rr T C x C x -+=⋅⋅=,()51x -的展开式的通项公式为()5151kkk k T C x -+=⋅-⋅,则展开式中含4x 的项需满足4r k +=,∴展开式中4x 的系数为()()20413454511C C C C ⋅-+⋅-()()342231454511C C C C +⋅-+⋅-()540451C C +⋅-54060201=-+-+-6=-,故选:A .【点睛】本题主要考查二项展开式的通项公式的应用,考查计算能力与推理能力,属于中档题. 6.在三角形ABC 中,a ,b ,c 分别为角A ,B ,C 的对边,且满足22265b c a bc +=+,则sin 2B C +⎛⎫=⎪⎝⎭( )A.2C.5【答案】D 【分析】根据余弦定理结合题意得3cos 5A =,而sin cos 22B C A +⎛⎫= ⎪⎝⎭,再根据半角公式求解即可.【详解】解:∵22265bc a bc +=+,即22265a b c bc -=+,由余弦定理可得2222cos a b c bc A =+-, ∴62cos 5bc A bc =, ∴3cos 5A =,则02A π<<, ∵ABC π++=, ∴1cos 25sin cos 222B C A A ++⎛⎫===⎪⎝⎭, 故选:D .【点睛】本题主要考查余弦定理的应用,考查半角公式的应用,属于基础题.7.函数()32x e f x x -=-的部分图像大致是( )A. B. C. D.【答案】A 【分析】根据指数函数的值域和绝对值的几何意义可知()302x e f x x -=>-,再结合导数求出函数在()2,+∞上的单调性,由此可得出答案.【详解】解:根据指数函数的值域和绝对值的几何意义可知()302x e f x x -=>-,则C 、D 错; 当2x >时,()32x x e f x --=,()()()23'32x f x x e x ---=, 由()'0f x >得3x >,由()'0f x <得23x <<,∴函数()f x 在()2,3上单调递减,在()3,+∞上单调递增,则A 对,B 错;故选:A .【点睛】本题主要考查函数图象的识别,考查利用导数研究函数的单调性,属于中档题.8.已知函数()31663x x f x x x e e -=--+-,若211011f f a a ⎛⎫⎛⎫+≤ ⎪ ⎪+-⎝⎭⎝⎭,则a 的取值范围为( ) A. (][),12,-∞-⋃+∞ B. ()1,2-C.()()1,00,1-UD.()[)1,12,-+∞U【答案】D 【分析】先判断函数的奇偶性,再求导研究函数的单调性,再结合奇偶性与单调性解不等式即可.【详解】解:∵()31663x x f x x x e e -=--+-,定义域为R ,∴()()31663x x f x x x e f x e --=+-+=-, ∴函数()f x 为奇函数,∵()21660x x f'x x e e -=---<-,∴函数()f x 在R 上单调递减,∵211011f f a a ⎛⎫⎛⎫+≤ ⎪ ⎪+-⎝⎭⎝⎭ ∴21111f f a a ⎛⎫⎛⎫≤-⎪ ⎪+-⎝⎭⎝⎭,则21111f f a a ⎛⎫⎛⎫≤ ⎪ ⎪+⎝-⎝⎭⎭, ∴21111a a ≥+-, ∴()()2011a a a +-≥-, ∴()()20110a a a -≤⎧⎨+-<⎩或()()20110a a a -≥⎧⎨+->⎩,解得11a -<<,或2a ≥, 故选:D .【点睛】本题主要考查利用函数的奇偶性与单调性解不等式,考查利用导数研究函数的单调性,考查分式不等式的解法,属于中档题. 9.已知等差数列{}n a 满足:11a =,4164a a +=,则1912222a a a⨯⨯⋅⋅⋅⨯=( )A. 382B. 192C. 162D. 762【分析】根据等差数列的性质和求和公式可得()()1194161219191922a a a a a a a ++++⋅⋅⋅+==,由此可求出答案. 【详解】解:∵等差数列{}n a 满足:11a =,4164a a +=,∴()()119416121919193822a a a a a a a ++++⋅⋅⋅+===,∴191219123822222a a a a a a ++⋅⋅⋅+⨯⨯⋅⋅⋅⨯==,故选:A .【点睛】本题主要考查等差数列的性质的应用,考查等差数列的求和公式,属于基础题.10.已知椭圆()222210x y a b a b +=>>21y x =+相切,则a =( )A. 2D. 1【答案】D 【分析】由题意可得222b c e a a b c =⎪==⎨⎪⎪-=⎪⎩,解出即可.【详解】解:由题意有,以原点为圆心以椭圆短半轴长为半径的圆的方程为222x y b +=, 直线21y x =+的一般式为210x y -+=,又椭圆22221x y a b+=,∴222b c e a a b c =⎪==⎨⎪⎪-=⎪⎩,解得1a b c ⎧⎪=⎪⎪=⎨⎪⎪=⎪⎩,【点睛】本题主要考查椭圆的标准方程及其几何性质,考查直线与圆的位置关系,属于中档题.11.设()f x 为定义于[]1,1-上的偶函数,当[]0,1x ∈时,()12f x x =-,则方程()()22xf f x =的实数解的个数为( ) A. 8 B. 6C. 4D. 2【答案】A 【分析】由题意可知,()()f f x 为偶函数,()[]0,1f x ∈,则()()()12f f x f x =-141,02134,12x x x x ⎧-≤≤⎪⎪=⎨⎪-<≤⎪⎩,画出函数()()f f x 和22x y =在[]0,1上的图象,结合图象即可得出结论.【详解】解:当[]0,1x ∈时,()112,0212121,12x x f x x x x ⎧-≤≤⎪⎪=-=⎨⎪-<≤⎪⎩,∴()[]0,1f x ∈,∴当[]0,1x ∈时,()()()12f f x f x =-()()11212,0211221,12x x x x ⎧--≤≤⎪⎪=⎨⎪--<≤⎪⎩141,02134,12x x x x ⎧-≤≤⎪⎪=⎨⎪-<≤⎪⎩114,041141,421334,24343,14x x x x x x x x ⎧-≤≤⎪⎪⎪-<≤⎪=⎨⎪-<≤⎪⎪⎪-<≤⎩,又()()()()f f x f f x -=,∴()()ff x 为偶函数,且22x y =为偶函数,画出函数()()f f x 和22x y =在[]0,1上的图象如图,由图可知,函数()()f f x 和22x y =的图象在[]0,1上有4个交点,∴由偶函数的性质可知,函数()()f f x 和22x y =的图象在[]1,0-上有4个交点,∴函数()()f f x 和22x y =的图象在[]1,1-上有8个交点,即方程()()22x f f x =的实数解的个数为8,故选:A .【点睛】本题主要考查方程的根与函数的零点以及函数图象的交点之间的关系,考查转化与化归思想,考查分类讨论思想,考查数形结合思想,考查计算能力与推理能力,属于难题. 12.已知当[]0,1x ∈时,不等式()()22cos 11sin 0x x x x θθ--+->恒成立,则θ的取值范围为( )A. π5πππ1212k k θ+<<+(k 为任意整数) B. π5πππ66k k θ+<<+(k 为任意整数) C. π5π2π2π1212k k θ+<<+(k 为任意整数) D. π5π2π2π66k k θ+<<+(k 为任意整数) 【答案】C 【分析】可设不等式左边为()f x 并化简,求出()f x 的最小值,令其大于0,得到θ的取值范围即可.【详解】解:设()()22()cos 11sin f x x x x x θθ=--+-2(1sin cos )(2sin 1)sin x x θθθθ=++-++,①若1cos sin 0θθ++=,即3222k k θππππ=++或时,原不等式不恒成立; ②若1cos sin 0θθ++≠即3222k k θππππ≠++或时,()f x Q 在[0,1]的最小值为(0)f 或()1f 或2sin 1[]2(1cos sin )f θθθ+++,∴(0)0(1)02sin 1[]02(1cos sin )f f f θθθ⎧⎪>⎪⎪>⎨⎪+⎪>++⎪⎩, ∴sin 0cos 01sin 22θθθ⎧⎪>⎪>⎨⎪⎪>⎩,解得522()1212k k k Z πππθπ+<<+∈, 故选:C .【点睛】本题主要考查不等式恒成立的问题,考查三角函数的性质,考查分类讨论思想,考查计算能力与转化能力,属于难题.二、填空题:本大题共4小题. 13.设数列{}n a 满足14a=,210a =,215n a a -=,3n ∀≥,则201920181ln ln 2a a -=______. 【答案】ln 5 【分析】由题意可得,(()21lnln 5n n a a -=,化简整理得112112ln ln ln ln ln 522n n n n a a a a ---⎛⎫⎛⎫---= ⎪ ⎪⎝⎭⎝⎭,令11ln ln 2n n n b a a +=-,可得()122ln5ln5n n b b ---=-,由此可得ln 5n b =,从而可求出答案.【详解】解:∵215n a a -=,3n ∀≥,∴当3n ≥时,(()21lnln 5n n a a -=,即2112ln ln ln 52ln 2n n na a a --+=+, ∴1212ln 2ln ln ln 52n n n a a a ---+=,∴112112ln ln ln ln ln 522n n n n a a a a ---⎛⎫⎛⎫---= ⎪ ⎪⎝⎭⎝⎭, 令11ln ln 2n n n b a a +=-,则122ln 5n n b b ---=,且1211ln ln ln 52a b a =-=,∴()122ln5ln5n n b b ---=-,又1ln 50b -=, ∴ln 5n b =,即11lnln ln 52n n a a +-=, ∴201920181ln ln ln 52a a -=, 故答案为:ln 5.【点睛】本题主要考查数列递推公式的应用,考查推理能力与计算能力,考查转化与化归思想,属于中档题.14.设实数x ,y 满足02203x y x y x -≥⎧⎪++≥⎨⎪≤⎩,则22x y +的最大值为______.【答案】73 【分析】画出不等式组表示的可行域,利用目标函数的几何意义(到原点的距离的平方)转化求解即可. 【详解】解:不等式组的图象如图:22x y +的几何意义是可行域内的点和原点的距离的平方,显然A 到原点的距离最大,由2203x y x ++=⎧⎨=⎩,解得()3,8A -,则22xy +的最大值为:96473+=,故答案为:73.【点睛】本题主要考查线性规划的应用,利用目标函数的几何意义,通过数形结合是解决本题的关键,属于基础题.15.假设抛一枚质地均匀的色子,若抛出的点数为1、2或3,我们称为“小”,否则,若抛出的点数为4、5或6,则称为“大”.独立重复地抛这枚色子两次,已知两次都为“大”,则第1次抛出的点数为6的概率______. 【答案】13【分析】由题意可知,第1次抛出的点数为4、5或6,根据相互独立的事件的概率互不影响即可求出答案. 【详解】解:由题意可知,第1次抛出的点数为4、5或6, ∵独立重复地抛这枚色子两次,两次抛掷互不影响, ∴第1次抛出的点数为6的概率13p =, 故答案为:13. 【点睛】本题主要考查独立重复试验的应用,属于基础题. 16.已知定义于实数R 上的奇函数()f x 满足()'2f x >-,则不等式()()()2132ln 312f x x x x -<-+-的解集为______. 【答案】()0,1【分析】 设()()()()2132ln 312,0g x f x x x x x =----->,则()()()2132ln 312f x x x x -<-+-()0g x ⇔<,()()''14ln 46g x f x x x x =-+-+,令()4ln 46h x x x x =-+,则()'4ln h x x =,求导后可得()()12h x h ≥=,结合题意可得()'220g x >-+=,得函数()gx 在()0+∞,上单调递增,而()10g =,由此可求出解集. 【详解】解:设()()()()2132ln 312,0g x f x x x x x =----->,则()()()2132ln 312f x x x x -<-+-()0g x ⇔<,∵()()''14ln 46g x f x x x x =-+-+,令()4ln 46h x x x x =-+,则()'4ln h x x =,由()'0hx >得1x >,由()'0h x <得01x <<,∴当1x =时,函数()h x 取得极小值同时也是最小值()12h =,∵()'12fx ->-,()2h x ≥,∴()'220gx >-+=,∴函数()g x 在()0+∞,上单调递增, 又()()()()2111132ln13120gf =-----=, ∴由()0gx <得()()1g x g <,∴01x <<, 故答案为:()0,1.【点睛】本题主要考查利用导数研究函数的单调性,考查利用函数的单调性解不等式,考查计算能力与推理能力,属于难题.三、解答题:解答应写出文字说明、证明过程或演算步骤.第17~21题为必考题,每个试题考生都必须作答.第22、23题为选考题,考生根据要求作答. (一)必考题:17.设ABC V 中,()cos cos cos 0C A A B +=,内角A 、B 、C 对应的对边长分别为a 、b 、c .(1)求角B 的大小; (2)若2248a c +=,求ABC V 面积S 的最大值,并求出S 取得最大值时b 的值.【答案】(1)π3B =(2)面积S 的最大值为b =【分析】(1)在三角形中,()cos cos cos cos sin sin C A B A B A B =-+=-+,结合条件可得π2sin sin 03A B ⎛⎫-= ⎪⎝⎭,由此可求出答案;(2)由2248a c +=可得2ac ≤,则11sin 222S ac B =≤⋅=,此时2a =,1c =,再由余弦定理即可求出答案.【详解】解:(1)∵()cos coscos cos sin sin C A B A B A B =-+=-+,∴()cos cos cos sin cos cos C A A B A B A B +=-π2sin sin 03A B ⎛⎫=-= ⎪⎝⎭,∵sin 0A >,0πB <<,∴πsin 03B ⎛⎫-= ⎪⎝⎭,则π3B =; (2)因a ,0c >,2248a c +=,2244a c ac +≥,故2ac ≤,于是,11sin 22222S ac B =≤⋅⋅=,∴ABC V 面积S 的最大值为2, 且当S 取得最大值时,2ac =,2a c =,可得2a =,1c =,由余弦定理,2222cos 3b a c ac B =+-=,即得b =【点睛】本题主要考查余弦定理的应用,考查三角形的面积公式,考查重要不等式的应用,属于基础题. 18.如下为简化的计划生育模型:每个家庭允许生男孩最多一个,即某一胎若为男孩,则不能再生下一胎,而女孩可以多个.为方便起见,此处约定每个家庭最多可生育3个小孩,即若第一胎或前两胎为女孩,则继续生,但若第三胎还是女孩,则不能再生了.设每一胎生男生女等可能,且各次生育相互独立.依据每个家庭最多生育一个男孩的政策以及我们对生育女孩的约定,令X 为某一家庭所生的女孩数,Y 为此家庭所生的男孩数.(1)求X ,Y 的分布列,并比较它们数学期望的大小;(2)求概率()()PX D X >,其中()D X 为X 的方差.【答案】(1)分布列见解析:EX EY =(2)14【分析】 (1)易知X 的取值为0,1,2,3,Y 的取值为0,1,利用相互独立的事件的概率公式求出相应概率,由此可得分布列,再根据数学期望的计算公式求出期望,进而比较大小;(2)结合公式()()22)(D X E X EX =-求出方差,再根据互斥事件的概率加法公式即可求出结果. 【详解】解:(1)易知X 的取值为0,1,2,3,对应取值的概率为别为:()102P X ==,()211124P X ⎛⎫=== ⎪⎝⎭,()311228P X ⎛⎫=== ⎪⎝⎭,()311328P X ⎛⎫=== ⎪⎝⎭ 即得X 的分布列如下类似地,Y 的取值为0,1,对应取值的概率分别为:()()1038P Y P X ====,()()71108P Y P Y ==-==;得Y 的分布列如下:X0 1P18 78由X ,Y 的分布列可得它们的期望分别为:11117()012324888E X =⨯+⨯+⨯+⨯=,177()01888E Y =⨯+⨯=,因此()()E X E Y =;(2)()()22222221111771(012)32488864D XE X EX ⎛⎫=-=⨯+⨯+⨯+⨯-=⎪⎝⎭, 故()()()()71123644PX D X P X P X P X ⎛⎫>=>==+== ⎪⎝⎭. 【点睛】本题主要考查离散型随机变量的分布列及数学期望、方差,考查计算能力与推理能力,属于中档题. 19.如图,已知四棱锥P ABCD -的底面ABCD 为边长为2的菱形,PA ⊥平面ABCD ,2PA =,60ABC ∠=︒,F 为棱PC 上一点,且:1:3PF FC =.(1)求证:BD AF ⊥;(2)求二面角A PD C--余弦值;(3)求三棱锥F APD -的体积V . 【答案】(1)证明见解析;(2)77(3)36【分析】(1)由PA ⊥平面ABCD 得PA BD ⊥,又底面ABCD 为菱形可得AC BD ⊥,则BD ⊥平面PAC ,从而BD AF ⊥;(2)设菱形ABCD 的对角线交点为O ,以O 为原点,分别以OC u u u r 、OD uuu r的方向为x ,y 轴建立空间直角坐标系,借助空间向量求出平面法向量的夹角,从而求出答案;(3)由图可知P ACD F ACD V V V --=-,由题意可知三棱锥P ACD -的高为3342PA =,由此可求出答案. 【详解】解:(1)因PA ⊥平面ABCD ,故PA BD ⊥, 又因底面ABCD 为菱形,故AC BD ⊥,又PA AC A =I,,PA AC ⊂平面PAC ,∴BD ⊥平面PAC , 而AF⊂平面PAC ,∴BD AF ⊥;(2)设菱形ABCD 的对角线交点为O ,因AC BD ⊥,PA ⊥平面ABCD ,以O 为原点,分别以OC u u u r 、OD uuu r的方向为x ,y 轴建立如图所示的空间直角坐标系,则()1,0,0A-,()1,0,2P -,()3,0D ,()1,0,0C ,∴()0,0,2AP =u u u r,()3,0AD =u u u r ,()2,0,2PC =-u u u r,()3,0CD =-u u u r ,∴平面APD 和平面PCD 的一个法向量分别为()13,1,0r =-r,23,1,3r =u r,∴1212127cos ,27r r r r r r ⋅===⋅⨯r r r rr r ,由图可知二面角A PD C --的平面角为锐角, ∴二面角A PD C --7(3)由图可知,P ACD F ACD V V V --=-,因:1:3PF FC =,可知三棱锥P ACD -的高为3342PA =,∴1311232326ACD VS ⎛⎫=⨯⨯-==⎪⎝⎭△. 【点睛】本题主要考查线面垂直的判定与性质,考查二面角的求法,考查三棱锥的体积的求法,考查计算能力与推理能力,属于中档题.20.已知双曲线C :()222210,0x y a b a b-=>>的离心率3e =,其左焦点1F到此双曲线渐近线的距离为(1)求双曲线C 的方程; (2)若过点()2,0D的直线l 交双曲线C 于AB 两点,且以AB 为直径的圆E 过原点O ,求圆E 的圆心到抛物线24x y =的准线的距离.【答案】(1)2218y x -=(21+1【分析】(1)由题意可得2223c e a a b c ⎧==⎪=+=⎪⎩,解出即可; (2)由题意设直线AB的方程为2x my =+,联立直线与椭圆的方程并消元,设()11,Ax y ,()22,B x y ,可得韦达定理的结论,又以AB 为直径的圆过原点O 得12120x x y y+=,代入可求得2=±m ,根据中点坐标公式求得圆E 的圆心的纵坐标,从而可求出答案.【详解】解:(1)由题意可得2223c e a a b c ⎧==⎪=+=⎪⎩, 解得13a b c =⎧⎪=⎨⎪=⎩,∴双曲线C 的方程为2218y x -=;(2)易知直线AB 与x 轴不重合,设直线AB 的方程为2x my =+,联立方程22182y x x my ⎧-=⎪⎨⎪=+⎩,可得()228132240m y my -++=, 上述方程式的判别式()232830m ∆=+>,以及2810m -≠(否则直线l 不能与双曲线交两点), 设()11,Ax y ,()22,B x y ,则1223281m y y m +=--,1222481y y m =-, 同时可得()()()2212121212284222481m x x my my m y y m y y m +=++=+++=--,以AB 为直径的圆过原点O ,知12120x x y y +=,结合2810m -≠,可知28424m +=,=m , ∴圆E 的圆心即AB中点的纵坐标为12216281y y m m +=-=-, ∵抛物线24x y =的准线方程为1y =-,∴圆E 的圆心到抛物线24x y =的准线距离为1+1. 【点睛】本题主要考查椭圆的标准方程及其几何性质,考查直线与椭圆的位置关系,考查计算能力,考查转化与化归思想,属于中档题. 21.设函数()3ln aef x x b x=-+,0x >,其中e 为欧拉数,a ,b 为未知实数,且0a >.如果()0,e 和(),e ∞均为函数()f x 的单调区间.(1)求a ; (2)若函数()()3hx f x cx =+在()()0,,e e ⋃∞上有极值点,c 为实数,求c 的取值范围.【答案】(1)3a =(2)22,0,e e ⎛⎫⎛⎫-⋃∞ ⎪ ⎪⎝⎭⎝⎭【分析】(1)令()3ln 3ln ae aeg x xx x x=-=-,0x >,求导得函数()g x 在()0,∞上单调递增,设()0g x =的唯一根为0x ,则0x 满足003ln aex x =,由题设得0x e =, 由此可得答案; (2)由题意得存在()()00,,x e e ∈⋃∞,使得()0h x '=,再分类讨论结合一元二次方程根的分布即可求出答案.【详解】解:(1)令()3ln 3ln ae aeg x xx x x=-=-,0x >, ∴()23)0aeg x x x'=+>(因0a >,0x >), ∴函数()gx 在()0,∞上单调递增,设()0gx =的唯一根为0x ,即0x 满足003ln ae x x =,(利用3ln x ,ae x的函数图象很容易确定) 于是,当()00,x x ∈时,()0g x <,而当()0,x x ∈∞时,()0g x >, 从而,当()00,x x ∈时,()3ln aef x x b x =-+, 当()0,x x ∈∞时,()3ln aef x x b x=-+, 可知,()00,x 为()f x 的单调递减区间,()0,∞x 为()f x 的单调递增区间,进而,由题设得0x e =, 因此,003ln 3x x a e==; (2)若函数()()3hx f x cx =+在()()0,,e e ⋃∞上有极值点,则易知存在()()00,,x e e ∈⋃∞,使得()0h x '=,注意到()()()22333,0,333,,e c x e x xh x e c x e x x ⎧--+∈⎪⎪=⎨⎪++∈'∞⎪⎩,①若23330e c x x --+=在()0,e 上有根,等价于20ey y c +-=在1,e ⎛⎫∞ ⎪⎝⎭上有解,由一元二次方程根的分布可得,只需满足2110e c e e⎛⎫+-< ⎪⎝⎭,解得2c e >; ②若23330e c x x ++=在(),e ∞上有根,等价于20ey y c ++=在10,e ⎛⎫⎪⎝⎭上有解, 由一元二次方程根的分布可得,只需满足0c <且2110e c e e⎛⎫++> ⎪⎝⎭,解得20c e -<<;综上,c 的取值范围为22,0,e e ⎛⎫⎛⎫-⋃∞ ⎪ ⎪⎝⎭⎝⎭. 【点睛】本题主要考查利用导数研究函数的单调性,考查分类讨论思想,考查计算能力与推理能力,属于难题. (二)选考题:共10分.请考生在第22,23题中任选一题作答,如果多做,则按所做的第一题计分.作答时请写清题号.[选修4-5:不等式选讲] 22.设()f x 为定义于()0,1上的函数,满足:(1)对任意()0,1x ∈,都有()0f x >;(2)对任意x ,()0,1y ∈,都有()()()()121f x f x f y f y -+≤-. 求证:()f x 在()0,1上的导数恒为零.【答案】证明见解析; 【分析】由题意可得,对任意x ,()0,1y ∈,都有()f x ,()1f x -,()f y ,()10f y ->, 由()()()()121f x f x f y f y -+≤-得()()()()()()1121f x f y f y f x f y f y -+-≤-,令1x y=-,可得()()()()()()221210f x f x f x f x +---≤,即()()()210f x f x --≤,则()()1f x f x =-,于是()()()()121f x f x f y f y -+≤-化为()()22f x f y ≤,即()()f x f y ≤,同理,亦有()()f y f x ≤,则()()f x f y =,由此得证.【详解】证明:要证明()f x 在()0,1上的导数恒为零,等价于证明()f x 在()0,1上恒为常数;因对任意()0,1x ∈,都有()0f x >,故对任意x ,()0,1y ∈,都有()f x ,()1f x -,()f y ,()10f y ->,对任意x ,()0,1y ∈,都有()()()()121f x f x f y f y -+≤-, 故有()()()()()()1121f x f y f y f x f y f y -+-≤-,因上式对于任意()0,1x ∈都成立,故令1x y =-,可得()()()()()()221210f x f x f x f x +---≤,即()()()210f x f x --≤,∴()()1f x f x =-,()0,1x ∀∈,于是,()()()()121f x f x f y f y -+≤-可化为()()22f x f y ≤,即()()f x f y ≤,x ∀,()0,1y ∈, 同理,亦有()()f y f x ≤,x ∀,()0,1y ∈, 因此,x ∀,()0,1y ∈,()()f x f y =,即得证()f x 在()0,1上恒为一个常数,∴()f x 在()0,1上的导数恒为零.【点睛】本题主要考查抽象函数的导数的应用,考查转化与化归思想,考查计算能力,属于难题. [选修2-2,推理与证明] 23.设数列{}n a 为非负实数列,且满足1220k k k a a a ++-+≥,11ki i a =≤∑,1k =,2,….求证:1220k k a a k +≤-<,1k =,2,….【答案】证明见解析; 【分析】先证10k k a a --≥,1k=,2,…,反证法,若存在某个01k ≥,使得001k k a a +<,则有从0k a 起,非负数列{}n a 单调递增,从而得出矛盾,得到假设不成立; 再证122kk a a k+-<,1k =,2,…,令10k k k b a a +=-≥,则1k k k a a b ++=,有题意可知1k k b b +≥,再由条件可得到123123k b b b kb ≥+++⋅⋅⋅+()()1122k k k k k b b +≥++⋅⋅⋅+=,由此即可证明. 【详解】证明:先证10k k a a --≥,1k=,2,…,21若存在某个01k ≥,使得001k k a a +<,则有000001122k k k k k a a a a a ++++≤-+<,即从0k a 起,非负数列{}n a 单调递增, ∴1kii a =∑将随着k 的增加而趋于正无穷,不可能永远小于等于1, 即与11k ii a =≤∑,1k =,2,…矛盾, 故10k k a a +-≥,1k=,2,…; 再证122k k a a k+-<,1k =,2,…, 令10k k k b a a +=-≥,1k =,2,…,则1k k k a a b ++=,由1220k k k a a a ++-+≥可知1k k b b +≥,1k=,2,…, 又因123411ki k i a a a a a a =≥=++++⋅⋅⋅+∑12342k b a a a a =++++⋅⋅⋅+12323k b b a a =+++⋅⋅⋅+123123k k b b b kb ka +=+++⋅⋅⋅++12323k b b b kb ≥+++⋅⋅⋅+,故有()()1231123122k k k k k b b b kb k b b +≥+++⋅⋅⋅+≥++⋅⋅⋅+=, ∴()2221k b k k k ≤<+,即证得122k k a a k +-<,1k =,2,…; 综上:1220k k a a k +≤-<,1k =,2,…. 【点睛】本题主要考查反证法证明不等式,考查数列的递推公式的应用,考查推理能力与计算能力,属于难题.。
清华大学中学生标准学术能力诊断性测试2020届高三5月测试理综试题 Word版含答案
中学生标准学术能力诊断性测试2020年5月测试7.化学与生活生产密切相关,下列说法正确的是A.我国海洋开发走向深蓝,大型舰船的底部常镶嵌锌块做正极,防止船底腐蚀B.补铁酱油中含有三价铁,可补充人体缺乏的铁元素C.采用“燃煤固硫”、“煤的气化液化”、“静电除尘”、“汽车尾气催化净化”等方法,提高了空气质量,使我们的生活环境更美好D.“歼-20”飞机上使用的碳纤维被誉为“新材料之王”,是我国打破其他国家技术垄断,自主研发和生产的一种新型有机高分子材料8.下列说法正确的是A.14C与12C 60互为同位素B.14C60与12C60是具有相同质子数的不同核素C.CH3COOH与HCOOCH3互为同素异形体D.硝基苯与互为同分异构体9.用N A表示阿伏伽德罗常数的值,下列叙述不正确的是A.常温常压下,0.1mol的Na2O和Na2O2,混合物中阴离子总数为0.1N AB.常温下,46g的NO2和N2O4混合气体中含有的氮原子数为N AC.标准状况下,22.4L C3H6分子中含有N A个碳碳双键D.100mL0.1 mol·L−1CH3COONa溶液中阴离子总数大于0.01N A10.下列实验操作及现象和结论均正确的是11.向10mL 0.2mol·L−1的KIO3 溶液中逐渐加入NaHSO3 粉末,生成的I2的物质的量随所加NaHSO3的物质的量变化如右图所示,下列说法不正确的是A.A点对应的I 的物质的量为1.0×10−3molB.0a:ab=5:1C.当溶液中的n(I2)=5×10−4mol时,加入NaHSO3的物质的量可能为2.5×10−3mol或4.5×10−3mol D.由ab段反应可知,氧化性I2>HSO3−12.A、B、C、D为石墨电极,E、F分别为短周期相邻的两种活泼金属中的一种,且E能与NaOH 溶液反应。
按图接通线路,反应一段时间,当电路中通过1.0×10−3mol电子时,下列叙述正确的是A.U型管中的D端先变红,b烧杯中的溶液会变蓝B.a烧杯中最多有0.127gI2(KI足量)生成,甲池中的质量会减少0.04g(CuSO4溶液足量)C.A极产生气体的体积为5.6mL,甲池溶液的pH值为2D.E电极附近的溶液无明显现象产生13.常温下,将0.1mol·L−1的NaOH溶液逐渐加到5mL0.1mol·L−1的一元酸HA溶液中,测得混合溶液的BG(BG=-lg)与所加NaOH的体积的变化关系如图所示。
2020清华测试5月-理综化学答案

中学生标准学术能力诊断性测试2020年5月测试理科综合-化学参考答案(一卷)一、选择题:本题共7小题,每小题6分,共42分。
在每小题给出的四个选项中,只有一项是符合题目要求的。
三、非选择题:共58分。
(一)必考题:共43分。
26.(14分)答案:(一)(1)硫酸太稀,SO 2易溶于水不利于SO 2逸出;硫酸太浓,电离出的氢离子浓度减小,反应生成SO 2速率变小(2分,答对1点得1分)①干燥气体 ②控制流速,使二氧化硫与氧气的比例约为2:1 ③使两种气体均匀混合(2分,答对1点得1分)(2)排尽残留在装置中的SO 2与SO 3,使其被充分吸收,使测得的结果更准确(1分)(3)BaSO 4(1分) 80100%80233aa b ⨯+ (2分)(二)(1)HSO 3Cl+H 2O =HCl+H 2SO 4,HCl 气体在湿空气中形成盐酸小液滴(2分)(2)控制浓盐酸的流量,使其缓慢流入浓硫酸中(1分) 液体飞溅(或爆沸)(1分)(3)①吸收剩余的气体 ②防止空气中水分进入E (2分,答对1点得1分)27.(14分)答案:(1)AlO 2-+CO 2+2H 2O =Al(OH)3↓+HCO 3-(不加↓不扣分,2分)洗涤(1分) 灼烧(或煅烧)(1分)(2)V 2O 3+ Na 2CO 33+CO 2↑(不加↑不扣分,不加条件扣1分,2分)(3)VO 2++ H 2O VO 3-+2H +(反过来写也得分)(2分)(4)6(2分) 使VO 2++ H 2O VO 3-+2H +正向移动,VO 2-更多地转化为VO 3+(1分)NH 4VO 3(1分)(5)2NH 4O 5+2NH 3↑ +H 2O ↑ (不加↑不扣分,不加条件扣1分,2分)28.(15分)答案:(1)CH 3OH(l)+32O 2(g)=CO 2(g)+2H 2O(l) △H =3b+4d-a-c -a+1.5b-c+2d)2(或 (2分) (2)① Cr 2O 72-+6Fe 2++14H +=2Cr 3++6Fe 3++7H 2O (2分) ②8.4(2分)(3)①30(2分) ②小于(1分) ③大于(2分)(4)①c (Na +)﹥c (HA -)﹥c (H +)﹥c (A 2-)﹥c (OH -)(2分)② -7(a-0.1)100.2-a⨯(2分) (二)选考题:共15分。
清华大学中学生标准学术能力诊断性测试2020届高三5月测试文科综合地理试题 Word版含解析

中学生标准学术能力诊断性测试2020年5月测试文科综合地理试卷一、选择题:在每小题给出的四个选项中,只有一项是符合题目要求的。
海水受温度、盐度、运动等因素的影响,结冰期通常比陆地水域结冰期短。
海水在结冰过程中析出盐分,其盐度大大降低,成为可利用的淡水资源。
我国渤海每年冬季结冰期较长,潜在可利用淡水资源达1000亿立方米。
下图为2019年2月渤海海冰分布。
据此完成下面小题。
1. 海冰最丰富的海域是A. ①B. ②C. ③D. ④2. 有人建议开发渤海海冰资源,理由可能是A. 开采海冰的成本较低B. 环渤海地区淡水资源缺乏C. 开采海冰的难度较小D. 海冰资源丰富且不易枯竭3. 大规模开发海冰资源产生的不利影响有A. 海水污染加重B. 海水盐度升高C. 海洋灾害加剧D. 生物多样性减少下图为某区域多年平均降雪量与雪期(从当年初雪日到次年终雪日的天数)空间分布。
该区域内丘陵区每年因融雪径流造成的土壤侵蚀较为严重。
据此完成下面小题。
4. 多年平均雪期与多年平均降雪量并不完全吻合,说明影响多年平均雪期的主要因素是A 地形 B. 下垫面 C. 气温 D. 人类活动5. M-N一线多年平均雪期明显弯曲的影响因素是A. 纬度B. 地形C. 海陆分布D. 人类活动6. 图示区域因融雪径流导致侵蚀最严重的季节为A. 春季B. 夏季C. 秋季D. 冬季在城市化进程中,城市规模不断扩大,城市建成区边缘村庄的土地逐渐由集体土地转变为国有土地。
这些村庄原本位于城市的周边地带,随着城市的扩张,村庄和城市的相对距离逐渐缩短。
当相对距离接近零时,村庄便成了“城边村”。
西辛置村(位置见下图)位于青岛胶州三里河街道办事处西北侧,处扬州路西端城乡结合部,西邻胶西镇,东至市中心仅7km,高速公路穿越,朱诸路和扬州路对外联系。
据此完成下面小题。
7. “城边村”现象反映的城市化主要标志是A. 农村地区转化为城市地区B. 农业人口转化为非农业人口C. 农业活动转化为非农业活动D. 农业用地转化为非农业用地8. 一般情况下,“城边村”承接城市的主要部门是A. 餐饮业B. 金融业C. 制造业D. 旅游业9. “城边村”面临的主要社会问题是A 外来人口多,社会秩序压力大 B. 规划不合理,基础设施落后C 居民受教育水平低,素质较低 D. 制造业集中,环境污染加剧下图为哈尼族梯田生态系统示意图,这一结构被文化生态学家盛赞为河流—梯田—村落—森林四度同构的人与自然高度协调的、可持续发展的、良性循环的生态系统。
2020年5月北京市清华大学中学生标准学术能力诊断性测试THUSSAT诊断性测试理综试题物理答案

(1 分)
(二)选考题:共 15 分。请考生从 2 道物理题中任选一题作答。如果多做,则按所做的第 一题计分。
33.[物理—选修 3-3] (15 分) 答案: (1)BDE(5 分,选对一个 2 分,选对 2 个 4 分,选对 3 个 5 分,错选一个扣 3 分。最低 0
分) (2)(10 分)
第3页 共 5 页
设木板的长度为
L,则
d
−
L
=
1 2
a2t12
○13
解得 L = 3.5m
○14
小物块离开
D
点后做平抛运动,竖直方向
2R
=
1 2
gt22
○15
水平方向 x = vDt2
○16
解得 x = 4m
○17
(1 分) (1 分) (1 分)
(1 分) (1 分) (1 分) (1 分) (1 分)
由于 x L ,故小物块不能落在木板上 ○18
第2页 共 5 页
力 能 术 学 准 标
小物块通过 D 点时,mg = m vD2
③
R
从
C
点至
D
点,由机械能守恒定律得:1 2
mvC 2
=
2mgR
+
1 2
mvD2
④
解得: R = 2m ⑤
(2)由动能定理得:
(F
−
1mg)s
=
1 2
mvB 2
⑥
由牛顿第二定律得:F − 2mg = ma1
⑦
2mg − 3(M + m)g = Ma2
⑧
设小物块从 B 点运动至 C 点所需时间为 t1,则 vC = vB + a1t1
清华大学中学生标准学术能力诊断性测试2020届高三5月(THUSSAT)英语试题 (含答案)

中学生标准学术能力诊断性测试2020 年 5 月测试英语试卷本试卷共150 分,考试时间100 分钟。
第一部分阅读理解(共两节,满分60 分)第一节(共15 小题;每小题 3 分,满分45 分)ASummer is that time of this year 2019 when everyone looks to escape from the boring activities of life. So bring your kids to join us to have fun in the summer camp.Outdoor Word Game:All you need to do is setting up many huge letters that can be easily moved. These letters will help kids spell out certain words. Teenagers would also love something like this as it gives them the opportunity to invest their time in learning new words.Spray Painting:To bring out the creativity in a person, an activity centered on spray painting is the perfect way to get those creative juices flowing. All you need to do is providing plain white T-shirts and some cans of colored spray paint. It will be quite surprising to see some of the original ideas that will flow from individuals.Outdoor Twister:A person will be in charge of monitoring the game and will call out colors which can be placed outdoors. Participants have the task of placing either their hands or legs on the same color. A fun and exciting game focuses on hand-eye coordination(协调).The Maze(迷宫):One of the funniest games is the hallway maze game where a maze is constructed from either toilet paper or strings of paper. The chosen participant is then asked to make his way through the maze within a limited time without touching paper. This is one of the best and entertaining summer camp ideas for kids and adults also.1.Which activity most probably needs some imagination?A.Outdoor Word Game. B.Spray Painting.C.Outdoor Twister. D.The Maze.2.Which of the following is TRUE according to the passage?A.The maze is made of toilet paper in The Maze.B.You have to spray juice on your T-shirt in Spray Painting.C.Outdoors Twister tests whether you are good at controlling your body movement.D.You have to bring some huge letters to play in Outdoor Word Games.3.Who is the article intended for?A.Parents. B.Children. C.Teachers. D.Teenagers.BWhat makes a person sexy? Looks, talent, public image and how we accordingly view their personality all play into why we find ourselves attracted to certain celebrities and public figures. Every year, People put it all together when they name their “Sexiest Man Alive”. This year, the magazine decided that United States musician John Roger Stephens, better known as John Legend, has the full package.The title comes following some impressive achievements for the 40-year-old. He is among the youngest people to win an Emmy, Grammy, Oscar and Tony award, and the first African-American man to win all four of them. With numerous awards and nominations, an Ivy-League degree, and a handsome face, Legend has gained huge popularity worldwide. However, his dedication to family life maybe what wins over many.After getting married to US model Chrissy Teigen in 2013, Legend is the father of one son and one daughter. He’s not shy about telling the world their love is permanent. On the inside of Legend’s arm, there is a tattoo that says “Chrissy Luna Miles”, the names of his wife and children. In fact, Legend has gained strength from his family since childhood. He still remembers the days when his family would gather around his grandmoth er’s piano to sing Christmas songs. This inspired his passion for music. His best-known single, All of Me, is a love song to his wife. When he performed it at the 2014 Grammy Awards, many were touched by his delicate voice and the deep love beneath. “The more you know someone, the more you’re inspired by your relationship,” Legend told LA Confidential Magazine. “You have to grow enough to be able to write that song with sincerity and authenticity.”Despite his onstage confidence, Legend had to work to become a legend. As a teenager, he was the smallest kid in class. “I was always less mature, less at ease socially. I had plenty of awkward times,” he told People. “Most of your teenage years, into your twenties, you’re still figuring out who you are, how you present yourself to the world ... Eventually, I think you get more at ease with yourself.” Indeed, knowing who you are and be yourself could be the real key to attractiveness.4.What plays the biggest part in making Legend popular worldwide?A.High-level education. B.Handsome look.C.Efforts put in family. D.Talent in music.5.What does the third paragraph mainly talk about?A.Legend’s hobby as a boy.B.Legend’s family members.C.The secret behind Legend’s success.D.Composing background of the song All of Me.6.What can you infer from the passage?A.Legend is proud of his success in music.B.Legend’s interest in music originates from his family.C.Legend is the first black singer who won the Grammy award.D.Legend received the title “Sexiest Man Alive” before he got married.7.What is essential to attractiveness according to Legend?A.Being more mature.B.Being true to yourself.C.Having onstage confidence.D.Having attractive appearance.CWhat makes people trustworthy? Do they always tell the truth? Do they always keep their promises? In the near future, voluntary blood donations might be used as a clue to prove someone’s trustworthiness.On Nov 19, the National Health Commission (NHC) and other departments jointly released a document urging the social credit system to include voluntary blood donations. An incentive mechanism(激励机制)is also recommended, to provide donors with more conveniences and rewards like preferential treatment in using public facilities or visiting government-run parks, China Daily reported. “We need to show we care about donors so they feel honored,” Liu Jiang, director of Beijing’s blood donation office, told CCTV.Indeed, donating blood is key to saving many lives. Due to blood’s very limited shelf life, regular blood donations are needed from a sufficient number of healthy people. This ensures that safe blood will be available whenever and wherever it is needed, according to the World Health Organization. However, the notice received mixed reviews on social media, with some people questioning whether it was necessary to include voluntary blood donation in the social credit system, according to the South China Morning Post. The system records things like a person’s career information, and payments for credit cards or other debt expenses are used to tell whether a person or company is trustworthy. People who cannot pay debts on time would have a worse credit score, resulting in more difficulties borrowing money from banks, reported the P eople’s Daily.Many Sina Weibo users believe that blood donations should be voluntary. They say it has little to do with social credit. Others doubt whether it is appropriate to reward this action because some people would donate blood for reasons other than helping save people’s lives. Guangming Daily commented that only acts closely linked to personal credit should be included in the social credit system. A voluntary blood donor might refuse to pay his or her debts, while someone who doesn’t donate blood might have a perfect credit record, it noted.8.What benefit can voluntary blood donors get according to the 2nd paragraph?A.Free park tour. B.Free treatment in hospitals.C.Running in government parks. D.Priority in using public facilities.9.Why are blood donations regularly needed?A.Blood can’t be stored for long.B.Some donated blood is not healthy.C.Fewer people are willing to donate blood.D.More and more people need blood to get treated.10.What can you learn from the last paragraph?A.Many blood donors refuse to pay his or her debts.B.Whoever donates blood will have a perfect credit record.C.It’s right to reward blood donators for they save people’s lives.D.Some people agree that voluntary blood donation shouldn’t be linked to social credit. 11.What’s the author’s attitude to using voluntary blood donations to prove trustworthiness?A.Positive. B.Objective. C.Indifferent D.Negative.DFive years ago, UK schools began to learn Shanghai’s math teaching methods on account of top scores produced by Chinese teenagers in a global academic evaluation. This test is called the Program for International Student Assessment (PISA), run by the organization for Economic Cooperation and Development (OECD). Taking around three hours on computers, it mainly focuses on 15-year-olds’ reading, math and science abilities. Earlier this month, the latest test results were published.Students from Beijing and Shanghai, as well as Jiangsu and Zhejiang provinces, outperformed students from the other 78 participating education systems in mathematics and science by a wide margin. In reading comprehension scores, only Singapore came close. Chinese mainland students first took the tests in 2009, with Shanghai topping the scores. The city claimed the top spot again in 2012.The PISA rankings aroused global interest in Chinese teaching methods and as a result, Britain increased exchanges with Chinese schools to improve its math education. Nearly 600 teachers from Britain and China, mainly from primary schools, have taken part in the exchange since 2014, and around 5,000 of Britain’s 16,000 primary schools have adopted the Shanghai math teaching method. In the latest PISA test, Chinese mainland students averaged 591 points in math —more than 100 points higher than the OECD average. British students averaged 502, jumping from 27th place three years ago to 18th in the math rankings.Philip Avery, director of education for the Bohunt Education Trust, UK, said, “We’ve benefited fromworking with Chinese partners for the high quality of lesson planning and teaching for memorization in China. Our Chinese partners have benefited from our approach to the development of character, focus on innovation and application of knowledge.”However, Zhang Xuanmin, who is in charge of the PISA project in Shanghai, points out that besides basic education, Chinese students still need to improve their social and emotional competencies.12.Which of the following is TRUE according to the passage?A.UK teenagers are poorly-educated.B.UK schools’ teaching methods are out of fashion.C.Teenagers in Shanghai got higher scores in PISA.D.Teenagers in Shanghai are the best students in the world.13.What does the underlined word mean in the 2nd paragraph?14.What’s probably the average math point of OECD in the latest PISA test?15.What can be indicated by Philip Avery’s words?A.The exchange program is a win-win situation.B.Chinese students still have a long way to go in education.C.UK schools get more benefits from the exchange program.D.UK teenagers have gained a lot of improvement in math learning.第二节(共 5 小题;每小题 3 分,满分15 分)根据短文内容,从短文后的选项中选出能填入空白处的最佳选项。
清华大学中学生标准学术能力诊断性测试2020届高三5月(THUSSAT)英语试题 (含答案)

中学生标准学术能力诊断性测试2020 年 5 月测试英语试卷本试卷共150 分,考试时间100 分钟。
第一部分阅读理解(共两节,满分60 分)第一节(共15 小题;每小题 3 分,满分45 分)ASummer is that time of this year 2019 when everyone looks to escape from the boring activities of life. So bring your kids to join us to have fun in the summer camp.Outdoor Word Game:All you need to do is setting up many huge letters that can be easily moved. These letters will help kids spell out certain words. Teenagers would also love something like this as it gives them the opportunity to invest their time in learning new words.Spray Painting:To bring out the creativity in a person, an activity centered on spray painting is the perfect way to get those creative juices flowing. All you need to do is providing plain white T-shirts and some cans of colored spray paint. It will be quite surprising to see some of the original ideas that will flow from individuals.Outdoor Twister:A person will be in charge of monitoring the game and will call out colors which can be placed outdoors. Participants have the task of placing either their hands or legs on the same color. A fun and exciting game focuses on hand-eye coordination(协调).The Maze(迷宫):One of the funniest games is the hallway maze game where a maze is constructed from either toilet paper or strings of paper. The chosen participant is then asked to make his way through the maze within a limited time without touching paper. This is one of the best and entertaining summer camp ideas for kids and adults also.1.Which activity most probably needs some imagination?A.Outdoor Word Game. B.Spray Painting.C.Outdoor Twister. D.The Maze.2.Which of the following is TRUE according to the passage?A.The maze is made of toilet paper in The Maze.B.You have to spray juice on your T-shirt in Spray Painting.C.Outdoors Twister tests whether you are good at controlling your body movement.D.You have to bring some huge letters to play in Outdoor Word Games.3.Who is the article intended for?A.Parents. B.Children. C.Teachers. D.Teenagers.BWhat makes a person sexy? Looks, talent, public image and how we accordingly view their personality all play into why we find ourselves attracted to certain celebrities and public figures. Every year, People put it all together when they name their “Sexiest Man Alive”. This year, the magazine decided that United States musician John Roger Stephens, better known as John Legend, has the full package.The title comes following some impressive achievements for the 40-year-old. He is among the youngest people to win an Emmy, Grammy, Oscar and Tony award, and the first African-American man to win all four of them. With numerous awards and nominations, an Ivy-League degree, and a handsome face, Legend has gained huge popularity worldwide. However, his dedication to family life maybe what wins over many.After getting married to US model Chrissy Teigen in 2013, Legend is the father of one son and one daughter. He’s not shy about telling the world their love is permanent. On the inside of Legend’s arm, there is a tattoo that says “Chrissy Luna Miles”, the names of his wife and children. In fact, Legend has gained strength from his family since childhood. He still remembers the days when his family would gather around his grandmoth er’s piano to sing Christmas songs. This inspired his passion for music. His best-known single, All of Me, is a love song to his wife. When he performed it at the 2014 Grammy Awards, many were touched by his delicate voice and the deep love beneath. “The more you know someone, the more you’re inspired by your relationship,” Legend told LA Confidential Magazine. “You have to grow enough to be able to write that song with sincerity and authenticity.”Despite his onstage confidence, Legend had to work to become a legend. As a teenager, he was the smallest kid in class. “I was always less mature, less at ease socially. I had plenty of awkward times,” he told People. “Most of your teenage years, into your twenties, you’re still figuring out who you are, how you present yourself to the world ... Eventually, I think you get more at ease with yourself.” Indeed, knowing who you are and be yourself could be the real key to attractiveness.4.What plays the biggest part in making Legend popular worldwide?A.High-level education. B.Handsome look.C.Efforts put in family. D.Talent in music.5.What does the third paragraph mainly talk about?A.Legend’s hobby as a boy.B.Legend’s family members.C.The secret behind Legend’s success.D.Composing background of the song All of Me.6.What can you infer from the passage?A.Legend is proud of his success in music.B.Legend’s interest in music originates from his family.C.Legend is the first black singer who won the Grammy award.D.Legend received the title “Sexiest Man Alive” before he got married.7.What is essential to attractiveness according to Legend?A.Being more mature.B.Being true to yourself.C.Having onstage confidence.D.Having attractive appearance.CWhat makes people trustworthy? Do they always tell the truth? Do they always keep their promises? In the near future, voluntary blood donations might be used as a clue to prove someone’s trustworthiness.On Nov 19, the National Health Commission (NHC) and other departments jointly released a document urging the social credit system to include voluntary blood donations. An incentive mechanism(激励机制)is also recommended, to provide donors with more conveniences and rewards like preferential treatment in using public facilities or visiting government-run parks, China Daily reported. “We need to show we care about donors so they feel honored,” Liu Jiang, director of Beijing’s blood donation office, told CCTV.Indeed, donating blood is key to saving many lives. Due to blood’s very limited shelf life, regular blood donations are needed from a sufficient number of healthy people. This ensures that safe blood will be available whenever and wherever it is needed, according to the World Health Organization. However, the notice received mixed reviews on social media, with some people questioning whether it was necessary to include voluntary blood donation in the social credit system, according to the South China Morning Post. The system records things like a person’s career information, and payments for credit cards or other debt expenses are used to tell whether a person or company is trustworthy. People who cannot pay debts on time would have a worse credit score, resulting in more difficulties borrowing money from banks, reported the P eople’s Daily.Many Sina Weibo users believe that blood donations should be voluntary. They say it has little to do with social credit. Others doubt whether it is appropriate to reward this action because some people would donate blood for reasons other than helping save people’s lives. Guangming Daily commented that only acts closely linked to personal credit should be included in the social credit system. A voluntary blood donor might refuse to pay his or her debts, while someone who doesn’t donate blood might have a perfect credit record, it noted.8.What benefit can voluntary blood donors get according to the 2nd paragraph?A.Free park tour. B.Free treatment in hospitals.C.Running in government parks. D.Priority in using public facilities.9.Why are blood donations regularly needed?A.Blood can’t be stored for long.B.Some donated blood is not healthy.C.Fewer people are willing to donate blood.D.More and more people need blood to get treated.10.What can you learn from the last paragraph?A.Many blood donors refuse to pay his or her debts.B.Whoever donates blood will have a perfect credit record.C.It’s right to reward blood donators for they save people’s lives.D.Some people agree that voluntary blood donation shouldn’t be linked to social credit. 11.What’s the author’s attitude to using voluntary blood donations to prove trustworthiness?A.Positive. B.Objective. C.Indifferent D.Negative.DFive years ago, UK schools began to learn Shanghai’s math teaching methods on account of top scores produced by Chinese teenagers in a global academic evaluation. This test is called the Program for International Student Assessment (PISA), run by the organization for Economic Cooperation and Development (OECD). Taking around three hours on computers, it mainly focuses on 15-year-olds’ reading, math and science abilities. Earlier this month, the latest test results were published.Students from Beijing and Shanghai, as well as Jiangsu and Zhejiang provinces, outperformed students from the other 78 participating education systems in mathematics and science by a wide margin. In reading comprehension scores, only Singapore came close. Chinese mainland students first took the tests in 2009, with Shanghai topping the scores. The city claimed the top spot again in 2012.The PISA rankings aroused global interest in Chinese teaching methods and as a result, Britain increased exchanges with Chinese schools to improve its math education. Nearly 600 teachers from Britain and China, mainly from primary schools, have taken part in the exchange since 2014, and around 5,000 of Britain’s 16,000 primary schools have adopted the Shanghai math teaching method. In the latest PISA test, Chinese mainland students averaged 591 points in math —more than 100 points higher than the OECD average. British students averaged 502, jumping from 27th place three years ago to 18th in the math rankings.Philip Avery, director of education for the Bohunt Education Trust, UK, said, “We’ve benefited fromworking with Chinese partners for the high quality of lesson planning and teaching for memorization in China. Our Chinese partners have benefited from our approach to the development of character, focus on innovation and application of knowledge.”However, Zhang Xuanmin, who is in charge of the PISA project in Shanghai, points out that besides basic education, Chinese students still need to improve their social and emotional competencies.12.Which of the following is TRUE according to the passage?A.UK teenagers are poorly-educated.B.UK schools’ teaching methods are out of fashion.C.Teenagers in Shanghai got higher scores in PISA.D.Teenagers in Shanghai are the best students in the world.13.What does the underlined word mean in the 2nd paragraph?14.What’s probably the average math point of OECD in the latest PISA test?15.What can be indicated by Philip Avery’s words?A.The exchange program is a win-win situation.B.Chinese students still have a long way to go in education.C.UK schools get more benefits from the exchange program.D.UK teenagers have gained a lot of improvement in math learning.第二节(共 5 小题;每小题 3 分,满分15 分)根据短文内容,从短文后的选项中选出能填入空白处的最佳选项。
2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(理)试题(一卷)(wd无答案)

2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(理)试题(一卷)一、单选题(★) 1. 已知集合,,则()A.B.C.D.(★) 2. 在复平面内,复数的虚部为()A.B.C.D.(★) 3. 已知单位向量,满足,则()A.1B.2C.3D.4(★) 4. 下列程序框图的算法思想源于我国古代数学名著《九章算术》中的“更相减损术”.执行该程序框图,若输入,,则程序中需要做减法的次数为()A.6B.5C.4D.3(★★★) 5. 在的展开式中,的系数为()A.B.6C.10D.4(★★) 6. 在三角形中,,,分别为角,,的对边,且满足,则()A.B.C.D.(★★★) 7. 函数的部分图像大致是()A.B.C.D.(★★★) 8. 已知函数,若,则的取值范围为()A.B.C.D.(★★) 9. 已知等差数列满足:,,则()A.B.C.D.(★★★) 10. 已知椭圆的离心率为,以原点为圆心以椭圆短半轴长为半径的圆与直线相切,则()A.2B.C.D.1(★★★★) 11. 设为定义于上的偶函数,当时,,则方程的实数解的个数为()A.8B.6C.4D.2(★★★★) 12. 已知当时,不等式恒成立,则的取值范围为()A.(为任意整数)B.(为任意整数)C.(为任意整数)D.(为任意整数)二、填空题(★★★) 13. 设数列满足,,,,则______.(★★) 14. 设实数,满足,则的最大值为______.(★) 15. 假设抛一枚质地均匀的色子,若抛出的点数为1、2或3,我们称为“小”,否则,若抛出的点数为4、5或6,则称为“大”.独立重复地抛这枚色子两次,已知两次都为“大”,则第1次抛出的点数为6的概率______.(★★★★) 16. 已知定义于实数上的奇函数满足,则不等式的解集为______.三、解答题(★) 17. 设中,,内角、、对应的对边长分别为、、.(1)求角的大小;(2)若,求面积的最大值,并求出取得最大值时的值.(★★★)18. 如下为简化的计划生育模型:每个家庭允许生男孩最多一个,即某一胎若为男孩,则不能再生下一胎,而女孩可以多个.为方便起见,此处约定每个家庭最多可生育3个小孩,即若第一胎或前两胎为女孩,则继续生,但若第三胎还是女孩,则不能再生了.设每一胎生男生女等可能,且各次生育相互独立.依据每个家庭最多生育一个男孩的政策以及我们对生育女孩的约定,令为某一家庭所生的女孩数,为此家庭所生的男孩数.(1)求,的分布列,并比较它们数学期望的大小;(2)求概率,其中为的方差.(★★★) 19. 如图,已知四棱锥的底面为边长为2的菱形,平面,,,为棱上一点,且.(1)求证:;(2)求二面角的余弦值;(3)求三棱锥的体积.(★★★) 20. 已知双曲线:的离心率,其左焦点到此双曲线渐近线的距离为.(1)求双曲线的方程;(2)若过点的直线交双曲线于两点,且以为直径的圆过原点,求圆的圆心到抛物线的准线的距离.(★★★★) 21. 设函数,,其中为欧拉数,,为未知实数,且.如果和均为函数的单调区间.(1)求;(2)若函数在上有极值点,为实数,求的取值范围.(★★★★) 22. 设为定义于上的函数,满足:(1)对任意,都有;(2)对任意,,都有.求证:在上的导数恒为零.(★★★★) 23. 设数列为非负实数列,且满足,,,2,….求证:,,2,….。
THUSSAT中学生标准学术能力测试诊断性测试2020年5月测试测试清华大学文科数学一卷试题参考答案

−
4,
= −16k 2 +16 0,
所以 AB = 1+ k 2 x1 − x2 = 1+ k 2 16 −16k 2 ,
………………8 分
2k 2 + 2
又因为点 N 到直线 AB 的距离为 d =
,
1+ k2
………………10 分
所以△ NAB 的面积
( )( )( ) S = 4 1− k2 k2 +1 = 2 2 2 − 2k2 1+ k2 1+ k2
………………2 分
又因为 f (1) = 0 ,所以 f ( x) 在 x = 1处的切线方程 y = 2x − 2 ; …………4 分
(2)证明:当 k 2 时,函数 y = f (x) 的图象与直线 l 交点的个数等价于函数
h ( x) = ln x − k ( x −1) 的零点个数,
x +1
k
k
(2)因为直线 AB 经过点 M (t,1) ,直线 AB 的斜率为 k ,
所以可得直线 AB 的方程是: y = kx − 2k2 +1,
由
x2 = 4 y = kx
y, −
2k
2
+1,
联立可得 x2 − 4kx + 8k2 − 4 = 0,
x1 + x2 = 4k,
所以
x1
x2
=
8k 2
1 h 1 BC PB = 1 PO 1 BC DC ,
32
3
2
所以 h = 3 . 2
………………12 分
20.解:设点 A( x1, y1 ) , B ( x2 , y2 ) ,中点 M (t,1) ,
THUSSAT中学生标准学术能力测试诊断性测试2020年5月测试测试清华大学理科综合一卷试题参考答案

或: 若子代无低产植株,D 基因在 7 号正常染色体上 若子代出现低产植株,D 基因在 7 号异常染色体上
CH3
(3)
SO3H
CH3
SO3H (2 分,每个 1 分)
SO3H
避免苯环上对位的氢原子也被硝基取代使产物不纯(或减少副产物也得分)(1 分)
(4)
(2 分)
(5)13 (2 分) (6)
(2 分,写错 1 步扣 1 分)
第2页 共2页
案 答 考
术 学
参准
标
生
学
中
中学生标准学术能力诊断性测试 2020 年 5 月测试
洗涤(1 分) 灼烧(或煅烧)(1 分)
(2)V2O3+ Na2CO3+O2 = 煅烧 2NaVO3+CO2↑(不加↑不扣分,不加条件扣 1 分,2 分) (3)VO2++ H2O VO3-+2H+(反过来写也得分)(2 分) (4)6(2 分) 使 VO2++ H2O VO3-+2H+正向移动,VO2-更多地转化为 VO3+(1 分)
100%
(2 分)
80 233
(二) (1)HSO3Cl+H2O=HCl+H2SO4,HCl 气体在湿空气中形成盐酸小液滴(2 分) (2)控制浓盐酸的流量,使其缓慢流入浓硫酸中(1 分) 液体飞溅(或爆沸)(1 分) (3)①吸收剩余的气体 ②防止空气中水分进入 E(2 分,答对 1 点得 1 分) 27.(14 分) 答案: (1)AlO2-+CO2+2H2O=Al(OH)3↓+HCO3-(不加↓不扣分,2 分)
2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(文)试题(一卷)解析

绝密★启用前2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(文)试题(一卷)学校:___________姓名:___________班级:___________考号:___________注意事项:1、答题前填写好自己的姓名、班级、考号等信息 2、请将答案正确填写在答题卡上一、单选题1.已知集合{}{}14,2P x x Q x x =-<<=<,那么()R P C Q ⋂=()A .[)2,4B .()1,-+∞C .[)2,+∞D .(]1,2- 答案:A {}2R C Q x x =≥,所以()[)2,4R P C Q ⋂=,选A.2.已知复数z 满足4z i i =-(其中i 为虚数单位),则z 的虚部为() A .4iB .4C .1D .1- 答案:B把已知等式变形,再由复数代数形式的乘除运算化简得答案.解: 由4z i i=-,得2(4)414z i i i i i =-=-=+. ∴复数z 的虚部是4.故选:B .点评:本题考查复数代数形式的乘除运算,考查复数的基本概念,是基础题.3.已知实数x ,y 满足约束条件222y x y x ≤⎧⎪+≥⎨⎪≤⎩,则3x y +的最大值为()A .2B .6C .8D .12答案:C先根据约束条件画出可行域,再利用几何意义求最值,3z x y =+表示直线在y 轴上的截距,只需求出可行域直线在y 轴上的截距最大值即可.解:先根据约束条件画出可行域,作直线30x y +=,讲直线30x y +=平移,当过点(2,2)时,3x y +取得最大值 ()max 33228x y +=⨯+=故选:C .点评:本题主要考查线性规划问题,以及利用几何意义求最值,属于基础题.4.已知实数a ,b 满足5a b +=,23log log a b =,则ab =()A .2B .3C .5D .6 答案:D设23log log a b k ==,则2k a =,3k b =,再利用5a b +=即可求出k 的值,进而求出a ,b 的值.解:设23log log a b k ==,则2k a =,3k b =,235k k a b ∴+=+=,1k ∴=,2a ∴=,3b =,∴236ab =⨯=故选:D .点评:本题主要考查了对数式与指数式的互化,以及对数的运算性质,是基础题.5.已知向量a r ,b r 满足1a =r ,2a b -=r r 且a b ⊥r r ,则向量a r 与a b -r r 的夹角为()A .2π3B .π3C .5π6D .π6答案:B根据()a b b -⊥r r r 即可得出()0a b b -=r r r g ,从而得出·1a b =r r ,进而得出1cos ,2a b <>=r r ,根据向量夹角的范围即可求出夹角.解:Q a b ⊥r r ,∴0a b ⋅=r r∴()21a a b a a b ⋅-=-⋅=r r r r r r 设向量a r 与a b -r r 的夹角为θ∴()1cos 2a a b a a b θ⋅-==-r r r r r r ∵()0,θπ∈∴3πθ=故选:B .点评:考查向量垂直的充要条件,以及向量数量积的运算,向量夹角的余弦公式,属于中档题.6.已知函数()ln f x x x =,则函数()f x 的单调递增区间为()A .RB .()0,∞+C .1,e ⎛⎫+∞ ⎪⎝⎭D .(),e +∞答案:C 求()ln f x x x =的导数()f x ',由()0f x '>,即可求得答案.解:()ln 1f x x '=+Q ,令()0f x '>得:ln 1x >-,11x e e-∴>=. ∴函数()ln f x x x =的单调递增区间为1,e ⎛⎫+∞ ⎪⎝⎭. 故选:C .点评:本题考查利用导数研究函数的单调性,易错点在于忽视函数的定义域,属于中档题.7.数列{}n a 的前n 项和()2*23n S n n n =-∈N ,若5p q +=()*,p q ∈N ,则p q a a +=()A .6B .8C .9D .10答案:D 当1n =时,可得1a ,当2n …时,1n n n a S S -=-,验证1n =时是否适合可得通项公式,代入通项公式求解可得结果.解:当1n =时,11231a S ==-=-,当2n …时,221232(1)3(1)45n n n a S S n n n n n -=-=---+-=-, 当1n =时,上式也适合,∴数列{}n a 的通项公式为:45n a n =-∴()454541010p q a a p q p q +=-+-=+-=故选:D .点评:本题考查等差数列的前n 项和公式和通项公式的关系,属中档题.8.已知x ,y ∈R ,“1x y +≤且1x y -≤”是“1x y +≤”的()A .充分不必要条件B .必要不充分条件C .充要条件D .既不充分也不必要条件答案:C作出不等式组对应的平面区域,利用区域关系结合充分条件和必要条件的定义进行判断即可.解: 1x y +≤且1x y -≤等价于1111x y x y -≤+≤⎧⎨-≤-≤⎩ 1x y +≤等价于()()()()10,010,010,010,0x y x y x y x y x y x y x y x y ⎧+≤≥≥⎪-≤≥≤⎪⎨-+≤≤≥⎪⎪--≤≤≤⎩作出两个不等式组对应的平面区域都是以()1,0,()0,1,()1,0-,()0,1-为顶点的正方形∴“1x y +≤且1x y -≤”是“1x y +≤”的充要条件,故选:C .点评:本题主要考查充分条件和必要条件的判断,结合充分条件和必要条件结合平面区域的关系是解决本题的关键.9.已知实数0a ≠,则函数()1sin f x ax a ⎛⎫=+ ⎪⎝⎭的图象一定不可能的是() A .B .C .D .答案:A求函数的导数,判断()0f '的正负情况,即可得出答案.解:∵()1sin f x ax a ⎛⎫=+ ⎪⎝⎭∴()1cos f x a ax a ⎛⎫'=+ ⎪⎝⎭ ∴()10cos f a a'=, 观察各选项的图象,判断()0f '的正负情况,得:观察A 选项的图象,得()10sin 0f a=>,34T <<,故234a π<< ∴223a ππ<<,3122a ππ<<,()10cos 0f a a'=> 故A 选项的图象不符合观察B 选项的图象,得()10sin 0f a=>,67T <<,故267a π<< ∴273a ππ<<,3172a ππ<<,()10cos 0f a a'=> 故B 选项的图象符合观察C 选项的图象,得()10sin 0f a=>,69T <<,故269a π<< ∴293a ππ<<,3192a ππ<<,()10cos 0f a a'=> 故C 选项的图象符合观察D 选项的图象,得()10sin 0f a=>,24T <<,故224a π<< ∴2a ππ<<,112a ππ<<,()10cos 0f a a'=> 故D 选项的图象符合故选:A.点评:本题考查了函数的图象的识别,利用导数判断函数的性质,属于中档题.10.已知x ,y ∈R ,则方程组21y =⎪=+⎩的解(),x y 的个。
清华大学中学生标准学术能力诊断性测试2020年5月诊断性测试英语答案

中学生标准学术能力诊断性测试2020 年5 月测试英语参考答案1-5 BCACC 6-10 BBDAD 11-15 BCDBA 16-20 CGAEF21-25 ACDBC 26-30 BDADB 31-35 CCBAD 36-40 DAACB41.truly 42.themselves 43.that/which 44.based 45.was called46.friends 47.Another 48.how 49.to convey 50.knew短文改错We’ve all experienced positive and negative mood. The emotions we feel every day play amoods animportant role in how you think and behave. For one thing, people who are often in a positive moodwein their everyday life tends to be happier, healthier and get along better with others. For other, a badtend anothermood can ruin our day, as well the day of the people around us. However, there are many things weascan do to turn our bad mood into a good one. As far as I’m concerning, exercise is one of the keys toconcernedgetting rid from a bad mood. Meanwhile, the simple act of sharing time in others is a great way to release of withstress and anxiety. So next time we’re feeling down, and call up a friend or visit a neighbor.书面表达Dear editor,I’m so honore d to introduce the great changes that have taken place in my hometown for the last ten years. Ten years ago, my hometown was a small and poor city, which had fewer factories. And people had very limited choices on the daily diets and transportation. However, nowadays my hometown has taken on a brand-new look with rapid development of culture and economy. People have confidence when walking on the clean and busy street. Also, there are so many tall buildings standing around the city. When it comes to the transportation, private cars can be seen easily. Besides, people can have dinner in various restaurants.From my point of view, I really appreciate the life at present and I do believe our city will become more and more prosperous.Thank you for your reading.Yours,Li Hua。
2020年5月清华大学2020届高三毕业班中学生标准学术能力诊断性测试英语试题及答案

绝密★启用前2020年5月清华大学2020届高三毕业班中学生标准学术能力诊断性测试英语试题本试卷共150 分,考试时间100 分钟。
第一部分阅读理解(共两节,满分60 分)第一节(共15 小题;每小题 3 分,满分45 分)ASummer is that time of this year 2019 when everyone looks to escape from the boring activities of life.So bring your kids to join us to have fun in the summer camp.Outdoor Word Game:All you need to do is setting up many huge letters that can be easily moved. These letters will help kids spell out certain words. Teenagers would also love something like this as it gives them the opportunity to invest their time in learning new words.Spray Painting:To bring out the creativity in a person, an activity centered on spray painting is the perfect way to get those creative juices flowing. All you need to do is providing plain white T-shirts and some cans of colored spray paint. It will be quite surprising to see some of the original ideas that will flow from individuals.Outdoor Twister:A person will be in charge of monitoring the game and will call out colors which can be placed outdoors. Participants have the task of placing either their hands or legs on the same color. A fun and exciting game focuses on hand-eye coordination(协调).The Maze(迷宫):One of the funniest games is the hallway maze game where a maze is constructed from either toilet paper or strings of paper. The chosen participant is then asked to make his way through the maze within a limited time without touching paper. This is one of the best and entertaining summer camp ideas for kids and adults also.1.Which activity most probably needs some imagination?A.Outdoor Word Game. B.Spray Painting.C.Outdoor Twister. D.The Maze.2.Which of the following is TRUE according to the passage?A.The maze is made of toilet paper in The Maze.B.You have to spray juice on your T-shirt in Spray Painting.。
2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(文)试题(一卷)(wd无答案)

2020届清华大学中学生标准学术能力诊断性测试高三5月测试数学(文)试题(一卷)一、单选题(★★★) 1. 已知集合,那么()A.B.C.D.(★★) 2. 已知复数满足(其中为虚数单位),则的虚部为()A.B.4C.1D.(★★) 3. 已知实数,满足约束条件,则的最大值为()A.2B.6C.8D.12(★★) 4. 已知实数,满足,,则()A.2B.3C.5D.6(★★) 5. 已知向量,满足,且,则向量与的夹角为()A.B.C.D.(★★) 6. 已知函数,则函数的单调递增区间为()A.B.C.D.(★★) 7. 数列的前项和,若,则()A.6B.8C.9D.10(★★) 8. 已知,,“ 且”是“ ”的()A.充分不必要条件B.必要不充分条件C.充要条件D.既不充分也不必要条件(★★★) 9. 已知实数,则函数的图象一定不可能的是()A.B.C.D.(★★★)10. 已知,,则方程组的解的个数()A.0B.1C.2D.4(★★★) 11. 已知中,角,,所对的边分别是,,.若,且,则()A.B.C.或D.不存在(★★★)12. 已知,,,若三次函数有三个零点,,,且满足,,则的取值范围是()A.B.C.D.二、填空题(★★) 13. 已知和是椭圆的两个焦点,则______.(★★) 14. 将1名同学和2名老师随机地排成一排,则该名学生恰好在2名老师中间的概率为______.(★★★)15. 定义在上的偶函数满足,则______.(★★★★) 16. 已知中,,,.如图,点为斜边上一个动点,将沿翻折,使得平面平面.当______时,取到最小值.三、解答题(★★★) 17. 如图,点是锐角的终边与单位圆的交点,逆时针旋转得,逆时针旋转得,…,逆时针旋转得.(1)若的坐标为,求点的横坐标;(2)若点的横坐标为,求的值.(★★) 18. 某高中某班共有40个学生,将学生的身高分成4组:平频率/组距,,,进行统计,作成如图所示的频率分布直方图.(1)求频率分布直方图中的值和身高在内的人数;(2)求这40个学生平均身高的估计值(同一组中的数据用该组区间的中点值为代表)(精确到0.01).(★★★)19. 如图,四棱锥中,是边长为2的等边三角形.梯形满足:,∥ ,.(1)求证:;(2)若,求点到平面的距离.(★★★) 20. 如图,已知抛物线:,过直线上一点作直线交抛物线于,两点,且点为中点、作直线交轴于点.(1)求点的坐标;(2)求面积的最大值.(★★★★) 21. 已知函数.(1)求在处的切线方程:(2)已知实数时,求证:函数的图象与直线:有3个交点.(★★★) 22. 在极坐标系中,已知曲线:,过点引倾斜角为的直线,交曲线于,两点.(1)求曲线的直角坐标方程;(2)若直线分别交直线于,两点,且、、成等比数列,求的值.(★★★) 23. 已知实数,满足:.(1)求证:;(2)若对任意的,,恒成立,求的取值范围.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
中学生标准学术能力诊断性测试2020年5月测试
历史-文综参考答案
一、选择题(每小题4分)
二、非选择题
41.(25分)
(1)英国:投资数额不断增长,比例较稳定。
(2分)原因:固有的经济实力;重视投资的传统政策;侵华最早,势力范围最广。
(2分)
日本:投资数额和比例增长迅猛。
(2分)原因:经济实力逐步增强;邻近中国的优势;日俄战争胜利,抢夺俄国在华权益;不断扩大对中国的侵略。
(2分)
俄国:投资数额和比例均呈下降趋势。
(2分)原因:经济实力相对较弱;日俄战争失败优势下降;十月革命后政局变动。
(2分)
(2)1949年—70年代末:不侵犯受援国的主权利益;脱离国情,超越国力。
(3分)
70年代末—21世纪初:平等互利,共同发展;立足国情,实事求是。
(3分)
2012年至今:倡导建立人类命运共同体;彰显大国的责任和担当。
(3分)
(要求:明确划分阶段,说明阶段特征。
阶段有误酌情扣分。
)
(3)近代列强依仗不平等条约的特权,在华大肆掠夺,新中国对外援助坚持平等互利,不干涉内政;列强在华投资打压了中国的民族经济,新中国对外援助推动了受援国经济发展,加强了友好交往。
(4分)42.(12
评分标准:观点2分;论证8分;结论2分。
①观点明确,表述清晰;(2分)观点表述不准确或没有观点。
(0分)
②能对观点进行逻辑严密的论证,多角度多史实论证充分;(8-6分)逻辑较严密,论证较充分;(5-3分)逻辑不严密,缺乏史实或史实错误。
(2-0分)
③结论有理论高度,不是简单重复观点;(2分)结论错误或没有结论。
(0分)
示例:观点:我同意观点二,20世纪初是世界现代史的开端。
论证:随着第二次工业革命的深入,20世纪初,世界市场最终形成,由此,列强也掀起了瓜分世界的狂潮。
第一次世界大战后,国际力量对比发生巨大变化,凡尔赛-华盛顿体系重建了欧洲与亚洲的政治经济格局,世界历史进入一个新的阶段。
随着俄国十月革命的胜利和苏俄的建立,人类历史上第一次出现了崭新的社会主义生产方式和与之相适应的社会主义社会。
从此,资本主义和社会主义两种制度的并存及其相互影响和斗争,深刻影响了世界历史进程。
综上,世界近代史是西欧主导世界的历史,20世纪初开始,国际格局发生重大变化,世界步入现代史。
45.(15分)
(1)实行中等教育的分科制;设立专门学校;设立大学院校;建立职业教育系统。
(8分)
(2)推动中国教育的近代化;促进近代民族资本主义的发展;推进科技教育的普及和科学技术的发展;推动科技教育与国际接轨。
(7分,任答3点即可)
46.(15分)
(1)欧洲局势日趋紧张,战火可能向东蔓延;《慕尼黑协定》签订后,西方绥靖政策达到顶峰;苏联避战自保;警惕西方祸水东引;英、法、苏谈判陷入僵局;德国竭力拉拢苏联。
(8分,任答4
(2)为苏联赢得了宝贵的备战时间;同时使苏联放松警惕,导致卫国战争初期遭受严重的损失;使二战初期德国避免两线作战,助长了希特勒的侵略野心,加速了第二次世界大战的爆发;苏德划定分界和东欧势力范围,严重损害了弱小国家的利益和主权。
(7分,任答3点即可)
47.(15分)
(1)宋重文轻武,军备废弛;周边少数民族强势;宋夏矛盾尖锐;宋战略失误,屡战屡败。
(6分)(2)策略:坚持持久防御战,营田固城、选将练兵;怀柔周边少数民族,对夏和议。
(4分)
评:加强了宋朝的防御力量,减少战争伤亡和物资消耗;促进了民族团结;维护了宋朝的统治。
(5分)。
