White dwarf stars in D dimensions
跟天文知识有关的英语作文

跟天文知识有关的英语作文全文共3篇示例,供读者参考篇1The Wonders of the CosmosEver since I was a young child, I have always been fascinated by the night sky. I vividly remember lying in the backyard on warm summer nights, gazing up at the twinkling stars in awe and wonder. My parents would point out the constellations to me - Orion the Hunter, the Big Dipper, and more. Even at that young age, I was captivated by the vastness of the universe and the idea that those tiny pinpricks of light were enormous balls of gas millions of miles away.As I grew older, my curiosity about space only deepened. I devoured books on astronomy, mesmerized by the tales of ancient astronomers like Galileo and Copernicus who revolutionized our understanding of the cosmos. I learned about the birth and death of stars, the foreign landscapes of alien planets, and the mysteries that still baffle scientists to this day, like dark matter and black holes.In school, astronomy quickly became my favorite subject. While my friends zoned out during lessons on planetary motion and the life cycles of stars, I was hanging on every word. I loved learning about how stars are formed from massive clouds of dust and gas, burning bright for billions of years before eventually running out of fuel and collapsing in on themselves. Some go out with a whimper, shrinking into dense white dwarfs. But the largest stars meet a more spectacular demise, exploding in a brilliant supernova before their remnants form either a neutron star or a black hole from which not even light can escape.I found the concept of black holes particularly mind-bending. These gravitational behemoths, with their powerful tidal forces capable of spaghettifying any object that strays too close, really put into perspective the puniness of humanity in the grand scheme of things. At the same time, their sheer strangeness sparked my imagination. What lies beyond the event horizon, the point of no return? Is it possible to travel through a black hole's wormhole and emerge in another part of the universe? Or do they lead, as some scientists theorize, to entirely separate realities or dimensions?Learning about faraway exoplanets orbiting distant stars stirred my sense of wonder as well. For most of human history,we assumed our solar system was the only one of its kind. But in recent decades, scientists have identified thousands of exoplanets using cutting-edge telescopes and detection methods. Some are similar to the rocky inner planets like Earth and Mars, while others are gas giants akin to Jupiter and Saturn. But we've also discovered planets utterly unlike anything in our cosmic backyard, from scalding hot Jupiters orbiting precariously close to their suns to diamond planets crystallized by intense heat and pressure. Each new discovery deepens the mystery of how these strange worlds formed and whether any could potentially harbor life.On a cloudless night, I'll often set up my telescope and spend hours scanning the skies, hunting for distant galaxies and stellar phenomena. I've witnessed meteor showers that lit up the heavens like celestial fireworks displays. I've spotted wispy nebulae where new stars are being forged and crystal-clear globular clusters comprised of hundreds of thousands of ancient suns. The Andromeda Galaxy, our nearest major galactic neighbor, appears as a faint, fuzzy blob to the naked eye, but through my telescope's lens it transforms into a majestic spiral of glowing stars and dust.When I reflect deeply on how our sun is just one of billions of stars in the Milky Way, itself one of countless galaxies strewn across the vast ocean of the universe, my mind is overwhelmed by the immensity and age of it all. Our solar system, our galaxy, emerged from the ashes of the Big Bang nearly 14 billion years ago. The cosmos has been in a perpetual state of creation and destruction for eons beyond our comprehension. Stars are born, they live, they die, and from their remnants new ones eventually take form. This grand, unending cosmic ballet has played out over infinite time and space in a cycle as inexorable as it isawe-inspiring.To gaze upon the night sky and grasp, even for a moment, the epic scale of time and distance inherent in those ancient points of light is humbling in the most profound way. It lays bare our true insignificance in the fabric of the universe. For all our species' achievements and capabilities, we are but temporary wayfarers on a pale blue dot orbiting an utterly average star. We emerged from the cosmos, and to the cosmos we will one day return, our entire lineage a mere blip in the cosmic calendar.And yet, this existential perspective fills me not with dread, but an immense appreciation for the rarity and preciousness of our sliver of existence. We are profoundly fortunate to be here,alive, and able to look up on a clear night and bear witness to the majesty of creation. Out of the infinite cosmic void, we have developed the capacity to observe, to wonder, to have our souls stirred by the beauty and splendor of something as simple as a starry sky. We are small, yes, but we areers capable of no less than experiencing and revering the very universe itself.So I continue to keep watching the skies, pushing the boundaries of my understanding while simultaneously celebrating the grand mystery of it all. Perhaps one day we'll uncover insights that will reveal some deeper cosmic truths and unravel timeless riddles. But I don't mind either way. Because in this interminable dance of celestial spheres, the greatest gift is simply the journey of being able to embrace the unknown.篇2The Wonders of the Night SkyEver since I was a little kid, I've been fascinated by the night sky. There's just something magical about looking up at the twinkling stars and planets on a clear night. It makes you feel so small and insignificant in the grand scheme of the universe, yet also filled with a sense of awe and wonderment.I can still vividly remember one night when I was about 8 years old. It was a warm summer evening, and my dad had taken me out to our backyard after bedtime to go stargazing. We laid out an old blanket on the grass and just gazed upwards into the inky blackness. My dad pointed out the constellations - Orion with his distinctive belt of three bright stars, the Big Dipper hanging like a ladle in the northern sky, and Cassiopeia looking like a W made of stars. I was completely transfixed.That night sparked my lifelong interest in astronomy. As I grew older, I devoured books about the planets, stars, galaxies, and the mind-boggling vastness of space. I learned that a light year, the distance light travels in a vacuum in one year, is almost 6 trillion miles. Our entire solar system, as huge as it seems to us, would be just a tiny speck from that distance away. And our Milky Way galaxy contains over 100 billion stars! The numbers and distances involved in the study of the cosmos are truly beyond human comprehension.In school, my favorite units were always the ones on astronomy. I loved learning about how stars are born from massive clouds of dust and gas, fusing hydrogen atoms into helium and radiating their brilliant light for millions or billions of years. Eventually, stars like our Sun will run out of fuel, expandinto a red giant that could potentially swallow the Earth, and then shrink down into a smoldering white dwarf. The Universe's largest stars have even more dramatic fates, exploding as supernovas so bright they can outshine entire galaxies for weeks at a time.I found the history of astronomy and space exploration just as fascinating as the science itself. Ancient cultures like the Babylonians, Greeks, and indigenous Americans all studied the night sky and devised complex systems for tracking the motions of the planets and stars across the heavens. When Galileo turned his new telescope towards the heavens in 1610, he revolutionized our understanding by glimpsing craters on the Moon, spots on the Sun, and moons orbiting Jupiter.The 20th century will forever be remembered as the era when humanity first sent machines and then people out into space. The early satellites, manned spaceflights and Moon landings captured the imagination of the entire world. I'll never forget the iconic words of Neil Armstrong as he took his first steps onto the lunar surface in 1969: "That's one small step for man, one giant leap for mankind." Just 40 years later, we had landed rovers on Mars and captured stunning images of that rusty red world's ancient river valleys and extinct volcanoes.Looking ahead, the future of space exploration and astronomy is mind-boggling. NASA's new James Webb Space Telescope has already begun capturing incredible images of some of the oldest and most distant galaxies in the observable universe, shortly after the Big Bang. New super-powerful ground-based telescopes may someday find definitive evidence of Earth-like planets orbiting other stars where life could potentially exist. And Elon Musk's SpaceX is working towards establishing permanent human settlements on Mars within our lifetimes. Who knows what other wonders await to be uncovered in the depths of space?For my part, I plan to keep pursuing my passion for astronomy throughout my education and hopefully even make a career out of it someday. There's still so much about the cosmos left to explore and understand. Whether I end up as a researcher studying dark matter and black holes, an engineer helping design new space telescopes and rockets, or a science writer sharing the majesty of the heavens with the public, I know the night sky will keep filling my life with a sense of infinite possibility.To me, the greatest miracle of astronomy is forcing us to confront our own tiny place in this incomprehensibly vastuniverse. As the pioneering cosmologist Carl Sagan wrote, "We are a way for the cosmos to know itself." The atoms that make up our bodies were literally forged in the nuclear furnaces of ancient stars. We are made of star-stuff, both intimately connected to the cosmos yet somehow able to study and understand it through science. That humbling realization is what astounds me most of all.篇3The Wonders of the Cosmos: An Astronomical JourneyEver since I was a young child, I have always been fascinated by the night sky. There was something magical about gazing up at the twinkling stars and the glowing moon that filled me with a sense of wonder and curiosity. As I grew older and began learning about astronomy, my enchantment with the cosmos only deepened. The universe is a vast, mysterious realm that continues to astound scientists and amateur stargazers alike with its grandeur and complexity.One of the first astronomical concepts that captured my imagination was the life cycle of stars. These celestial beacons, which appear as tiny pinpricks of light in the night sky, are actually massive, blazing spheres of gas undergoing continuouscycles of birth, life, and death. Stars are formed from massive clouds of dust and gas known as nebulae. Over millions of years, the gravitational forces within these clouds cause the material to condense and form a protostar. As the protostar continues to contract, its core becomes incredibly hot and dense, setting off the nuclear fusion reactions that mark the birth of a new star.A star's life is a delicate balance between the inward pull of gravity and the outward push of the nuclear fusion occurring in its core. For most of its life, a star will exist in a stable equilibrium, fusing hydrogen into helium and radiating energy in the form of light and heat. However, as the star ages and exhausts its supply of hydrogen fuel, it enters into the final stages of its life cycle.Depending on the star's mass, it may undergo various transformations, such as expanding into a red giant or even a supergiant. Massive stars may end their lives in spectacular fashion, exploding as supernovae and briefly outshining entire galaxies. The remnants of these stellar explosions can form exotic objects like neutron stars or black holes, whose intense gravitational fields warp the very fabric of space and time.Another aspect of astronomy that has always captivated me is the study of galaxies. These vast, gravitationally bound systems of stars, gas, dust, and dark matter are the buildingblocks of the universe. Our own Milky Way galaxy is a spiral galaxy, containing hundreds of billions of stars and spanning over 100,000 light-years in diameter. Yet, it is but one of countless galaxies that populate the observable universe.The sheer scale and diversity of galaxies are trulymind-boggling. Some, like elliptical galaxies, are smooth and featureless, while others, such as spiral galaxies, display intricate patterns of dust lanes and stellar nurseries. Some galaxies even exist in clusters, bound together by the immense gravitational forces at play. And at the heart of many galaxies, including our own, lie supermassive black holes, objects so dense that not even light can escape their gravitational pull.Beyond the realm of individual galaxies lies the cosmic web, the large-scale structure of the universe itself. This vast, interconnected network of galaxies, galaxy clusters, and filamentary structures is the result of the intricate interplay between matter and the mysterious forces of dark matter and dark energy. These unseen components, which make up the bulk of the universe's mass and energy, continue to perplex astronomers and challenge our fundamental understanding of the cosmos.One of the most profound realizations in modern astronomy is that the universe itself had a beginning – the Big Bang. This cataclysmic event, which occurred approximately 13.8 billion years ago, marked the birth of space, time, and all matter and energy in the observable universe. The afterglow of this primordial explosion, known as the cosmic microwave background radiation, is a powerful piece of evidence supporting the Big Bang theory and provides a glimpse into the earliest moments of the universe's existence.As our knowledge of the cosmos continues to expand, new frontiers of exploration emerge. The search for exoplanets, or planets orbiting stars other than our Sun, has become a major focus of modern astronomy. With the advent of powerful telescopes and advanced detection techniques, thousands of exoplanets have been discovered, ranging from gas giants to rocky, Earth-like worlds. The possibility of finding habitable exoplanets and the potential for extraterrestrial life has ignited the imaginations of scientists and the public alike.Moreover, the study of dark matter and dark energy, which together make up approximately 95% of the universe's total mass and energy, remains one of the greatest unsolved mysteries in modern cosmology. Unraveling the nature of theseelusive components could revolutionize our understanding of the fundamental laws of physics and the ultimate fate of the universe.As a student of astronomy, I am constantly in awe of the vast and complex universe we inhabit. From the intricate dance of celestial bodies within our own solar system to the cosmic choreography of galaxies and clusters on the grandest scales, the cosmos is a tapestry of wonder and mystery waiting to be explored.The pursuit of astronomical knowledge is not merely an academic endeavor but a profound journey of self-discovery and existential questioning. By studying the heavens, we gain a deeper appreciation for our place in the cosmos and the interconnectedness of all things. We are reminded of our own insignificance in the grand scheme of the universe, yet simultaneously elevated by the knowledge that we are part of something much larger and more wondrous than ourselves.As I continue my studies and gaze up at the night sky, I am filled with a sense of humility and awe. The universe is a vast, ever-evolving canvas, and we are but tiny brushstrokes in its grand cosmic tapestry. Yet, it is through our curiosity, our thirst for knowledge, and our unwavering pursuit of understandingthat we can unravel the mysteries of the cosmos, one star, one galaxy, one cosmic epoch at a time.。
木星和土星的英文诗句

木星和土星的英文诗句Jupiter and Saturn's Poetic VerseThe celestial dance of Jupiter and Saturn has captivated the imaginations of stargazers and poets alike for millennia. These two majestic gas giants, each with their own unique charms, have inspired countless works of art and literature across cultures and throughout history. In the realm of poetry, their splendor has been immortalized in verses that celebrate their grandeur, their mysteries, and the awe they inspire in all who gaze upon them.Jupiter the Mighty stands tall in the night sky, its swirling storms and vibrant hues a testament to the sheer power and scale of the cosmos. Its Great Red Spot, a perpetual hurricane larger than our own planet, is a symbol of the raw, untamed forces that shape the universe. Yet amidst this turbulence, Jupiter also exudes a sense of regal elegance, a proud and commanding presence that has drawn comparisons to the mightiest of ancient gods.One poet, captivated by Jupiter's majesty, pens these words "O mighty Jove, your crimson cloak unfurls, a tapestry of storms that shake the heavens. Your steady gaze, a beacon in the night, guidesus through the vastness of the skies." The author's reverence for this colossal world is palpable, each line evoking the awe and wonder inspired by this celestial giant.Saturn, in contrast, is a world of delicate beauty, its iconic rings a testament to the intricate dance of gravity and matter that governs the heavens. This ringed wonder, with its serene and contemplative aura, has long been a source of fascination for poets and philosophers alike. They see in its graceful form a reflection of the harmony and balance that underpins the very fabric of the universe.One such poet muses "O Saturn, your rings a shimmering veil, a celestial lace that whispers of mysteries untold. Your moons, like silent sentinels, guard the secrets of your realm, inviting us to ponder the rhythm of the cosmos." The poet's words capture the sense of tranquility and wonder that Saturn evokes, a world of subtle beauty that invites contemplation and deeper understanding.Yet these gas giants are not merely passive observers in the grand cosmic dance. They are dynamic, ever-changing worlds, their atmospheres and orbital patterns constantly in flux, responding to the gravitational interplay between them and the other denizens of the solar system. This interplay has inspired poets to explore the relationship between these two giants, weaving intricate tapestries of verse that capture the delicate balance and tension that existsbetween them.One such poet writes "Jupiter and Saturn, celestial partners in the eternal ballet, their gravitational embrace a symphony of celestial movements. They dance across the heavens, their rhythmic motions shaping the destiny of worlds, their influence felt throughout the solar system." The poet's words evoke a sense of the grand cosmic drama that unfolds between these two titans, a drama that has captivated the human imagination for eons.But the poetry inspired by Jupiter and Saturn is not limited to their physical forms and celestial interactions. These worlds have also come to symbolize deeper philosophical and spiritual truths, serving as metaphors for the great mysteries of existence. Poets have seen in these giants reflections of the human condition, with all its complexities, contradictions, and eternal questions.One poet, contemplating the vastness of these worlds, writes "Jupiter and Saturn, twin guardians of the cosmos, remind us of our smallness and our grandeur. In their endless dance, we see the rhythm of life and death, the cycle of creation and destruction that governs all things." The poet's words suggest that these giants, for all their immensity, also hold the keys to understanding our own place in the universe, our own significance and insignificance.Another poet, struck by the timeless quality of these worlds, pens these lines "Jupiter and Saturn, ageless sentinels of the night, bear witness to the rise and fall of empires, the birth and death of stars. They stand as monuments to the eternal, reminding us that our own fleeting existence is but a heartbeat in the grand symphony of the cosmos." The poet's words invoke a sense of humility and reverence, reminding us that we are but transient visitors in the grand scheme of the universe.Through the power of poetry, Jupiter and Saturn have been elevated to the realm of the mythic and the archetypal. They have become symbols of the great mysteries and truths that underlie the universe, touchstones for the human search for meaning and understanding. And in this, they continue to inspire and captivate, their celestial dance a source of endless fascination and wonder for all who gaze upon them.。
恒星

太阳
主序星
红巨星
红巨星(恒星的成熟阶段),在 红巨星(恒星的成熟阶段),在 ), 这恒星迅速膨胀的同时, 这恒星迅速膨胀的同时,它的外表面离中心 越来越远,所以温度将随之而降低, 越来越远,所以温度将随之而降低,发出的 光也就越来越偏红。不过,虽然温度降低了 光也就越来越偏红。不过, 一些,可红巨星的体积是如此之大, 一些,可红巨星的体积是如此之大,它的光 度也变得很大,极为明亮。 度也变得很大,极为明亮。肉眼看到的最 亮的星中,许多都是红巨星。 巨星的体 亮的星中,许多都是红巨星。红巨星的体 很大,它的半径一般比太阳 100倍 积很大,它的半径一般比太阳大100倍。
巨分子云( 巨分子云(GMC) )
直径:300光年 温度:20K 密度:100~300 cm-3 质量:106 M⊙ 寿命:10~100万年 像这样的巨分子云只有 10%的比较致密的可以 孕育原恒星
星际尘埃
成分 硅或石墨微粒, 外面被冰或二氧化碳包裹。 形成 形成于红(超)巨星的外层大气(低温→气体凝结) , 在恒星演化晚期被吹向星际空间。 星际尘埃与星际分子 分子云中的尘埃屏蔽了星光中的紫外线,使分子免遭瓦解, 尘埃有利于分子形成。
自然界中的黑洞是宇宙中最完美的宏观物 体: 构成它们的唯一要素是我们对空间和时间 的概念. 由于广义相对论只有唯一一组描写它 们的解, 因此它们又是宇宙中最简单的物体. -------Chandrasekhar
黑洞
黑洞是超巨星经过超新星爆发遗留的产物 大质量黑洞就是超巨黑洞,星系的中心, 大质量黑洞就是超巨黑洞,星系的中心, 质量是太阳的百万-十亿倍, 质量是太阳的百万-十亿倍,10 6-10M☼ 中等质量黑洞: 中等质量黑洞:100 M☼ 小质量黑洞,~10 相当太阳10 10倍左 小质量黑洞,~10 M☼, 相当太阳10倍左 ,~ 右的恒星发生超新星爆炸后形成
恒星的一生浙教版科学课件1

太阳
这是一个恒星
主序星
这些都是恒星,你认识吗? 这些都是恒星,你认识吗?
红巨星 超新星
行星状星云
白矮星
中子星
黑洞
白矮星:M4星 白矮星:M4星图中的白矮星 :M4 (White Dwarf Stars in Globular Cluster M4)
红巨星:哈勃望远镜所拍摄的参宿四(Betelgeuse) 巨星: 远镜所拍摄 宿四(Betelgeuse) 所拍
太阳
太 阳 系
太阳系的中心,质量占99.86% 太阳系的中心,质量占99.86% 类地 水、金、地、火 行星
木、土 天、海、冥 巨行星
九大行星
远日 行星
彗星
彗核、彗发、 彗核、彗发、彗尾
流星、流星群、 流星体 、流星、流星群、陨石等
“康德—拉普拉斯星云说” 康德—拉普拉斯星云说” 康德 太阳系是由一块星云收缩形成的, 太阳系是由一块星云收缩形成的, 先形成的是太阳,然后, 先形成的是太阳,然后,剩余的星云物 质进一步收缩演化,形成地球等行星。 质进一步收缩演化,形成地球等行星。
太阳
红巨星
暗矮星
白矮星
思考: 思考:
了解了太阳一生的演化之后,你对宇 了解了太阳一生的演化之后, 宙有了什么新认识。 宙有了什么新认识。 宇宙也像恒星一样,也有诞生,成长, 宇宙也像恒星一样,也有诞生,成长, 死亡的时候。 死亡的时候。
恒星的一生
通过天文观测和发现逐步证实和完善了恒星 的演化理论。 的演化理论。 星际气体 收缩形成 原恒星 白矮星 主序星 暗矮星 中子星 大恒星 超红巨星 超新星 黑洞
太阳 主序星
红巨星
恒星也有自己的生命史,它们从诞生、 恒星也有自己的生命史,它们从诞生、成 长到衰老,最终走向死亡。它们大小不同, 长到衰老,最终走向死亡。它们大小不同,色 彩各异,演化的历程也不尽相同。恒星与生命 彩各异,演化的历程也不尽相同。 的联系不仅表现在它提供了光和热。 的联系不仅表现在它提供了光和热。实际上构 成行星和生命物质的重原子就是在某些恒星生 命结束时发生的爆发过程中创造出来的。 命结束时发生的爆发过程中创造出来的。
IRIS梵高特辑之流转的星月夜通用PPT模板

”
0 单击此处2插入标题
星星和月亮的黄色使天空脱颖而出,将我们的眼睛吸引到有 着一个个漩涡的天空。
单击此处插入标题
01 单击此处插入您想展现的的内容 02 单击此处插入您想展现的的内容 03 单击此处插入您想展现的的内容
0 单击此处3插入标题
他曾说过:“我问自己,为何天空中的星星不能像法国地图 上的斑点那样触手可及,就像我们坐火车前往塔拉斯康或鲁 昂,或许我们死了就可以触到星星了。”
生动地描画了充满运动和变化的星空。夜晚的天空高又远,大星、小星回旋于夜空,金
黄的满月形成庞大的漩涡,星云的短线条纠结、盘旋,好像让人们看见时光的流逝。暗
绿褐色的柏树像庞大的火焰,是星夜狂欢的响应者。天空下,安睡的村庄那么XXX、安
详。淡蓝的色调,动感的线条,给人自由的时空感。
本文本采取微软雅黑字体,18号字,段落格式为首行缩进,1.5倍行距。
0 单击此处4插入标题
画中的树是柏树,是最常显现在墓地并且与死亡相关的植物, 它向天空延伸出去,好像就要触到星星一样。
单 击 此 处 插
01 入
您 想 展 现 的 的 内 容
单 击 此 处 插
02 入
您 想 展 现 的 的 内 容
单 击 此 处 插
03 入
您 想 展 现 的 的 内 容
单 击 此 处 插
流
梵 高
·转 的 星月源自夜汇报人 :XXX
目
01 单击此处插入标题
录
02 单击此处插入标题
03 单击此处插入标题
04 单击此处插入标题
0 单击此处1插入标题
安静的村落和螺旋的天空形成强烈的对照,体现了梵高内心 深处的不安,XXX融入天空,小村庄的每栋房屋都用黑色明 确的勾画出来。
Wave-particleduality
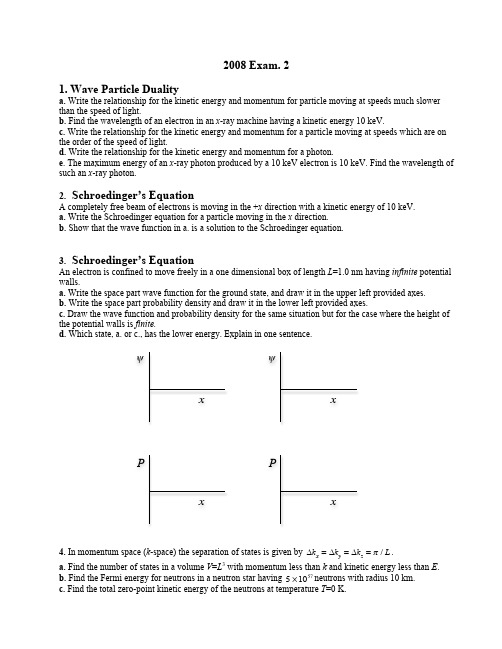
1. Wave Particle Dualitya. Write the relationship for the kinetic energy and momentum for particle moving at speeds much slower than the speed of light.b. Find the wavelength of an electron in an x -ray machine having a kinetic energy 10 keV.c. Write the relationship for the kinetic energy and momentum for a particle moving at speeds which are on the order of the speed of light.d. Write the relationship for the kinetic energy and momentum for a photon.e . The maximum energy of an x -ray photon produced by a 10 keV electron is 10 keV. Find the wavelength of such an x -ray photon.2. Schroedinger’s EquationA completely free beam of electrons is moving in the +x direction with a kinetic energy of 10 keV. a. Write the Schroedinger equation for a particle moving in the x direction. b. Show that the wave function in a. is a solution to the Schroedinger equation.3. Schroedinger’s EquationAn electron is confined to move freely in a one dimensional box of length L =1.0 nm having infinite potential walls.a. Write the space part wave function for the ground state, and draw it in the upper left provided axes.b. Write the space part probability density and draw it in the lower left provided axes.c. Draw the wave function and probability density for the same situation but for the case where the height of the potential walls is finite.d. Which state, a. or c., has the lower energy. Explain in one sentence.4. In momentum space (k -space) the separation of states is given by Δk x =Δk y =Δk z =π/L .a. Find the number of states in a volume V =L 3 with momentum less than k and kinetic energy less than E .b. Find the Fermi energy for neutrons in a neutron star having 5×1057neutrons with radius 10 km.c. Find the total zero-point kinetic energy of the neutrons at temperature T =0 K.ψx ψxP x P x1. R elativityA s tar i s e mitting l ight i n t he p ositive x d irection. T he w avelength o f t he l ight i s 400 n m.a. (5 p t) W hat i s t he p eriod Δt i n n s o f o ne o scillation o f l ight i n t he s tar’s f ixed reference f rame.Assuming t he w ave t urns o n a t t=0b. (5 p t) H ow f ar d oes i t g o i n t =100 n s i n t he s tar’s f ixed f rame?c. (5 p t) W rite t he 4-‐vector f or t he s pace-‐time p osition a fter a t ime 100 n s.d. (5 p t) O btain t he s pace-‐time i nvariant i nterval t hat t he l ight t ravels i n 100 n s. Suppose t he s tar m oves a way f rom t he e arth i n t he p ositive x d irection w ith a v elocity 0.8c.e. (5 p t) W hat i s t he p eriod Δ′t i n n s o f o ne o scillation o f l ight i n t he e arth’s m oving r eference f rame?f. (5 p t) H ow f ar d oes t he l ight t ravel a fter o ne o scillation a s s een b y t he e arth.f. (5 p t) W rite t he 4-‐vector f or t he s pace-‐time p osition a fter a t ime ′t corresponding t o one o scillation a s s een f rom t he e arth’s r eference f rame.g. (5 p t) O btain t he s pace-‐time i nvariant i nterval i n t he e arth’s f rame t hat t he l ight travels i n 100 n s2.) B ohr m odel.According t o t he B ohr m odel o f t he h ydrogen a tom, a n e lectron i n t he g round s tateorbits a t a r adius o f a bout 0.5 A o. S uppose t he e lectron i s r eplaced b y a m uon( mµc2=105 MeV) t o f orm a m uonic a tom.a. (10 p t) W hat i s t he r adius o f o rbit f or t he m uonic a tom i n i ts g round s tate?b. (10 p t) W hat a re t he e nergies o f t he g round a nd f irst a nd f irst e xcited s tates?c. (10 p t) W hat i s t he w avelength c orresponding t o t he t ransition b etween t he f irst exciteds tate a nd t he g round s tate?3.)Schroedinger e quation.A s imple h armonic o scillator (SHO) h as a m ass m a nd s pring c onstant K. T he p otential e nergy is 1/2Kx2.a. (10 p t) W rite t he S chroedinger e quation f or t he s pace p art o f t he S HO.b. (10 p t) T he w ave f unction f or t he g round s tate h as t he f orm Ae bx2. B y d irects ubstitution s how t his i s a s olution, a nd t hereby f inding t he c onstant b i n t erms o f m a nd Kc. (10 p t) W rite t he p robability d istribution f or t he g round s tate, a nd c arefullyg raph i t.d. (10 p t) W rite a n i ntegral w hichwould b e u sed t o o btain t he n ormalizing c onstantA.You d o n ot n eed t o s olve t his i ntegral)4.)Schroedinger E q. i n 3 d imensions.Consider a t hree d imensional c ubic p otential w ell w ith r igid (infinite) w alls, h avingsides o f d imension L x = L y = L z = L=0.1 n m.a. (5 p t) W rite t he S chroedinger e quation f or a p article w ithin t he w ell.b. (5 p t) W rite t he q uantum c onditions o n k x , k y a nd k z.c. (5 p t) O btain t he q uantum c ondition o n t he w ave n umber k2.d. (5 p t) O btain t he q uantum c ondition o n t he a llowed e nergies E.e. (5 p t) W rite t he g round s tate s olution Ψ(x,y,z)to t he S chroedingere quationf or a p article w ithin t he w ell.f. (5 p t) W rite t he p robability d ensity f or a p article w ithin t he w ell i n t he g roundstate.g. (5 p t) O btain t he n umerical r esult o n t he a llowed e nergies E i n u nits o f e V.h. (5 p t) O btain t he n umber o f e lectrons w hich c an b e a ccommodated a t e ach o ft he l owest 3 e nergy l evels. T ake i nto a ccount t hat d ifferent c ombinations o fq uantum n umbers c an h ave t he s ame e nergy, a nd t hat t wo e lectrons,c orresponding t o s pin u p a nd d own c an f it i ntoe ach c ombination of s patialq uantum n umbers.2006 Exam. 21. A b aby s eal i n t he p acific o cean h as a b ody t emperature o f 310 K. I f t he m ean temperature o f t he w ater i s 287 K a t w hat r ate w ill t he s eal l ose e nergy b y r adiating p hotons? (σ=5.7×10−8 W⋅m-2⋅K-4)2. W ave p article d uality.Compare t he w avelength a nd f requency o f a p hoton a nd e lectron, e ach w ith k ineticenergy 10 K eV.3. B ohr m odel.a. U se t he B ohr m odel o f t he a tom t o e stimate t he e nergy l evels o f p ositronium, i n w hich a n electron o rbits a p ositron.b. T he i onization e nergy (binding e nergy) o f a n e lectron i n h ydrogen i s 13.6 e V.What i s t he i onization e nergy o f p ositronium?4. P article i n a b ox.Approximate a n a tomic n ucleus a s a n i nfinite c ubical b ox o f s ide L=2 f m, w here1 f m = 10-‐15 m, i n w hich t he n ucleons m ove f reely.a. O btain a n e xpression f or t he w avelength o f t he g round, o r l owest l ying e nergy s tate.b. W hat i s t he k inetic e nergy o f a n eutron i n t he g round s tate o f t his a tom. T he r estenergy o f a n eutron i s m c2=939 M eV.5. S imple h armonic o scillator.A n a pproximate r epresentation o f t he i nteraction b etween t wo a toms i n a d iatomicm olecule i s a s pring l ike f orce F=-Kx w ith o scillator f requency ω=. T ake t he f orcec onstant t o b e 8×103 e V/nm2 = 1000 N/m, a nd t he m ass of e ach a tom a round t o b e5×10−27kg(mc2=4.69 G eV). T he w ave f unction f or t he g round s tate o f a s imple h armonic o scillatori s ψ0(x)=mωπ⎛⎝⎜⎞⎠⎟1/4e−mω2x2.a. W hat i s t he e nergy o f t he g round s tate?b. F ind t he w ave f unction i n m omentum s pace b y p erforming a F ourier t ransformation.6. D ensity o f s tates a nd F ermi e nergy.a. F ind t he a verage e nergy o f a n e lectron i n a w hite d warf s tar o f r adius 10,000 k mcontaining 2×1057 n ucleons, h alf o f w hich a re p rotons. T he d ensity o f s tates d istribution i sdNdE=E1/2.b. F rom t he r esults i n p art a, c omment o n w hether i t i s a pproporiate t o u se n on-‐relativistickinematics.Other p roblems f rom p revious e xams:1. A f ree e lectron h as k inetic e nergy 1000 e V. I t m oves i n t he x-y p lane i n a d irection w hichmakes a n a ngle 30 d eg. r elative t o t he x a xis.a. F ind i ts m omentum p, w avelength λ a nd w ave n umber k.b. W rite t he w ave f unction Ψ(x,y,z,t) i n s ymbols (not n umerical v alues) i n C artesiancoordinates.c. W rite t he p robability d ensity P(x,y,z).d. W hat c an y ou s ay a bout t he u ncertainty i n t he e lectron’s p osition.Approximate a n ucleus c onsisting o f f ree n ucleons i n a s pherical r igid w all p otential w ith radius R=4 f m. F or t he i sotope 17O:a. W hat a re t he q uantum n umbers o f e ach o f t he n eutrons a nd p rotons?b. W hat a re t he e nergies o f e ach o f t he n eutrons a nd p rotons i n t he i sotope 17O?2. a. W rite t he w ave f unction f or a f ree p article m oving i n 3-‐dimensional C artesiancoordinates.b. T he r elativistic v ersion o f t he S chroedinger e quation i s c alled t he K lein-‐Gordon e quation.Using E2=p2c2+m2c4, c onstruct t he K lein-‐Gordon e quation b y e xpressing t he e nergy a nd momentum i n t erms o f d ifferential o perators.c. S how t hat t he w ave f unction i n p art a. i s a s olution t o t he K lein-‐Gordon w ave f unction t hatwas c onstructed i n p art c.3.) C onsider a n e lectron w hich m oves f reely i n a 2 d imensional i nfinite s quare w ell o f s ide a . a. W rite t he S chroedinger e quation f or t his c ase. b. W hat a re t he a llowed v alues o fk x and k yc. W hat a re t he a llowed e nergy l evels?d. I f a =10Angstroms, w hat i s t he l owest e nergy.e. W rite t he w ave f unction f or t his s tate.4.) T he t hree p rimary t erms w hich d etermine t he b inding e nergy o f a n ucleusare v olume , s urface a nd C oulomb , E V , E S , E C e nergies.a. W hat i s t he R a nd Z d ependence o f e ach, w here R i s t he n uclear r adius a nd Z t he a tomic number.Also i ndicate t he s ign o f e ach. i E V ∝ii E S ∝iii E C ∝b. W hat i s t he A a nd Z d ependence o f e ach, w here A i s t he n umber o f n ucleons. A lso i ndicate the s ign o f e ach. i E V /A ∝ii E S /A ∝iii E C /A ∝c. D raw t he m agnitude o f e ach a s a f unction o f A , a s w ell a s t he s um o f e ach. B e s ure t o c learly fill i n t he e nergy s cale i n t he v ertical a xis a nd t he n umber o f n ucleons i n the h orizontal a xis a t t he p osition o f t he t ic m arks.5.) I n t he b lank s paces p rovided i n t he t able, f ill i n t he p roperties o f t he p article s hown, a s w ell a s t he energy s cales a nd q uark m akeup w here a ppropriate.6.Draw a g raph f or t he s hape o f t he n ucleon-‐nucleon a ttractive p otential e nergy, i ndicating the a pproximate r ange a nd d epth.particl e Charge Rest m ass energy Units o f energy QuarkFlavor c ontent p +1 .93 GeV uud nπ−.139 π+ e 0.511 ν γ W 89 g7. a . 92238U c aptures a n eutron, f ollowed b y a symmetric f ission i nto 2 u nbound n eutrons a nd3892Sr a nd 54140Xe . O btain t he d ifference i n t he b inding e nergy b etween t he i nitial 92238U and t he f inal 3892Sr a nd 54140Xe n uclides, a nd t herefore t he e nergy r eleased.b . C alculate t he k inetic e nergyd ue t o t he e lectrostatic r epulsion b etween t he 3892Sr a nd 54140Xe w hen t hey a re s till t ouching, a nd s how t hat i t i s t he s ame o rder a s y our a nswerin p art a . a bove. (note:r =r 0A 1/3with r 0≈1.2fm.)8.Fill i n t he t able b elow:9. a . T he m ajor s ource o f e nergy p roduction i n t he s un i s t he p roton-‐proton c ycle. Trace t he s teps o f t he p -p c ycle a s w e d iscussed i n c lass.b . I f t he f inal r esult i s t he f usion o f 4 p rotons i nto 4He ,c alculate t he t otal e nergy r eleased in t he c ycle.10. D raw a F eynman d iagram f or e ach o f t he f ollowing p rocesses, a nd i dentify t he e xchanged quantum:a. e - +µ+♑e - +µ+via t he e lectromagnetic i nteraction. b. e - +µ+♑e + +µ- v ia t he w eak i nteraction.c. u +u →s +s v ia t he s trong i nteraction.6. F rom t he i nformation o n s pin, b aryon n umber a nd s trangeness g iven i n t he t ablebelow, f ill i n t he q uark f lavor c ontent a nd d ecay i nteraction o f e ach o f t he f ollowing h adrons.Decay interact we。
2023-2024学年全国天域名校协作体高三下学期3月联考英语试题

2023-2024学年全国天域名校协作体高三下学期3月联考英语试题1. Why is the man phoning the woman?A.To ask her out for dinner.B.To tell her he will be late.C.To inform her he has been in an accident.2. What does the man like his toast with?A.Much butter. B.Less butter. C.Nothing on it.3. What is the man going to buy his father?A.A new digital camera. B.An antique desk. C.An old film camera.4. How fast did the man drive on the straightaway?A.180 km/h. B.200 km/h. C.220 km/h.5. How is the man feeling?A.Sad. B.Awkward. C.Proud.听下面一段较长对话,回答以下小题。
6. When was the last time the speakers were in a theater?A.January. B.March. C.July.7. What stage show did the speakers watch on television?A.Cats. B.Romeo and Juliet. C.Hamilton.听下面一段较长对话,回答以下小题。
8. Where does the conversation probably take place?A.In a bar. B.In a hotel. C.In a restaurant.9. How much is the barbecue?A.$10. B.$20. C.$30.10. What is the woman going to do first?A.Have a drink. B.Take a shower. C.Eat some food.听下面一段较长对话,回答以下小题。
白矮星的结构特点

2 极限质量
由上面的计算知,半径小的白矮星质量大,当半径最小时质量有最大值。现计算白矮星的极限质量。 当白矮星的质量大时半径小, 密度 ρ ≈ 1010 kg/m3, 中心温度 T ≈ 107 K, 而相应的费米温度 TF = 3 × 109 K, 白矮星上的电子气体是高度简并的,可看成是处在绝对零度时的理想费米气体。 dN [3](P258) 由 dN/dp 与 p 的关系曲线,可知在 p>pF 处 =0, 考虑到电子的自旋,则有: dp N = ∫ dN = V ⋅
0 pF ! 2 ρ 8πm p 2 8π pF 2 8πpF N ρ 3h3 3 [4](P358) = p , p d p = V 由 ρ = µ m 可得 p = ( ) F e p F µe 3h3 h3 ∫0 3h3 V µe 8πm p
[1](P301)
其中 µe = 压强 Pe =
2
度,因此白矮星的电子气体是高度简并的。电子的运动情况与白矮星的密度有密切关系,质量小的白矮 星平均密度 ρ ≤109kg/m3,简并电子运动远小于光速,是非相对论性的。质量大的白矮星,平均密度超 过 109kg/m3,简并电子的运动速度与光速相当,是相对论性的。因此白矮星内部电子运动的相对论程度取
r
r [3](P225) ), 半径为 r 的球的质量为: R
r 4 3 r4 4 3 3r )d r = π r ρ − ρ π = π r ρ c (1 − ) c c ∫0 R 3 R 3 4R R R 4 r 1 M = ∫ ρ ( r )d r = ∫ ρ c (1 − )4 π r 2 d r = ρ c π R 2 又因为 M = πR3 ρ ,因此可得 ρc = 4 ρ ,则: 0 0 3 3 R 4 π r 2 ρ c (1 − 16 3r π r 3 ρ (1 − ) 3 4R 16 3r r G π r 3 ρ (1 − )16 π r 2 ρ (1 − )d r R G M ( r )d m ( r ) 16 2 2 2 13 5 3 4R R R = −∫ = −∫ = −G π ρ 0 0 r r 3 840 3 M 2 16 × 13 × 5 3 GM 2 = − G = − × 1.24 5 R 840 5 R
英语介绍梵高星空的作文

英语介绍梵高星空的作文下载温馨提示:该文档是我店铺精心编制而成,希望大家下载以后,能够帮助大家解决实际的问题。
文档下载后可定制随意修改,请根据实际需要进行相应的调整和使用,谢谢!并且,本店铺为大家提供各种各样类型的实用资料,如教育随笔、日记赏析、句子摘抄、古诗大全、经典美文、话题作文、工作总结、词语解析、文案摘录、其他资料等等,如想了解不同资料格式和写法,敬请关注!Download tips: This document is carefully compiled by theeditor. I hope that after you download them,they can help yousolve practical problems. The document can be customized andmodified after downloading,please adjust and use it according toactual needs, thank you!In addition, our shop provides you with various types ofpractical materials,such as educational essays, diaryappreciation,sentence excerpts,ancient poems,classic articles,topic composition,work summary,word parsing,copyexcerpts,other materials and so on,want to know different data formats andwriting methods,please pay attention!Van Gogh's Starry Night is a masterpiece that captures the beauty and mystery of the night sky. The painting is filled with vibrant colors and swirling brushstrokes that create a sense of movement and energy.The first thing that strikes me about Starry Night is the intense blue color used for the sky. It is a deep, almost electric blue that seems to glow with its own light. The stars in the painting are depicted as bright yellow and white dots, scattered across the sky in a seemingly random pattern.As I look closer at the painting, I notice the small village that is nestled at the bottom of the canvas. The houses are painted in shades of blue and green, blending in with the surrounding landscape. The tall cypress tree in the foreground adds a sense of verticality to the composition, contrasting with the horizontal lines of the village.One of the most striking features of Starry Night is the swirling movement of the brushstrokes. Van Gogh used thick, bold strokes to create a sense of energy and dynamism. The sky seems to be in constant motion, with the stars and clouds swirling together in a mesmerizing dance.The use of color in Starry Night is also worth noting. Van Gogh used a wide range of colors, from the vibrant blues of the sky to the warm yellows and oranges of the stars and moon. The contrasting colors create a sense of harmony and balance in the painting.Starry Night is not just a representation of the night sky, but also a reflection of Van Gogh's inner emotions. The painting was created during a time when Van Gogh was struggling with mental illness, and it is said to reflect his feelings of loneliness and despair. The swirling brushstrokes and intense colors can be seen as a visual expression of his inner turmoil.In conclusion, Van Gogh's Starry Night is a mesmerizingpainting that captures the beauty and mystery of the night sky. The intense colors, swirling brushstrokes, and emotional depth make it a truly unique and captivating work of art.。
介绍梵高星空的英语作文80词

介绍梵高星空的英语作文80词Starry Night by Van Gogh is one of the most famous and iconic paintings in the world. This masterpiece was created in 1889 and is currently housed in the Museum of Modern Art in New York City. The painting depicts a small village with a towering steeple, surrounded by a peaceful countryside. The sky above is filled with swirling clouds and bright stars, which give the painting its name.Van Gogh's use of color in Starry Night is truly remarkable. The deep blues and vibrant yellows create a sense of movement and energy. The brushstrokes are bold and expressive, reflecting the artist's emotional state at the time. Van Gogh painted Starry Night while he was staying in a mental asylum in Saint-Rémy-de-Provence, and it is believed that the painting reflects his feelings of isolation and longing for freedom.One of the most striking elements of Starry Night is the way Van Gogh portrays the stars. The stars in the painting are not simply white dots in the sky; they are bright, swirling orbs that seem to dance across the canvas. Some art historians believe that Van Gogh was inspired by the scientific theories of the time, which suggested that the stars were actually swirling masses of energy. Others believe that Van Gogh was simply using his imagination to create a sense of movement and vitality in the painting.In addition to the stars, Van Gogh also includes a crescent moon in Starry Night. The moon is depicted as a bright yellow orb, casting a soft glow over the village below. The moon adds a sense of tranquility and serenity to the painting, contrasting with the vibrant colors and swirling brushstrokes of the sky.Starry Night has captivated audiences for over a century, and its popularity continues to grow. The painting has been the subject of countless reproductions, parodies, and reinterpretations. It has inspired musicians, poets, and filmmakers, who have sought to capture the beauty and emotion of Van Gogh's masterpiece in their own art.In conclusion, Starry Night is a truly remarkable painting that continues to captivate audiences with its vibrant colors, swirling brushstrokes, and imaginative depiction of thenight sky. Van Gogh's use of color and motion creates a sense of energy and emotion, while the inclusion of the stars and moon adds a sense of tranquility and serenity. Starry Night is a testament to Van Gogh's genius as an artist, and it will continue to inspire and awe audiences for generations to come.。
描写西方画作星空的英语作文

The Enchanting Starry Skies in Western Art Western art has a rich history of depicting the starry skies, and the paintings that feature the night's wonders have captured the hearts and minds of countless viewers. The allure of these paintings lies in their ability to transform the vast and often overwhelming expanse of the universe into a beautiful and accessible narrative.One of the earliest and most renowned examples of a starry sky painting is Vincent van Gogh's "Starry Night." Painted in 1889, this masterpiece captures the essence of the night sky in a way that is both realistic and abstract. Van Gogh's use of bold strokes and vivid colors creates a sense of movement and energy that seems to bring the stars and constellations to life. The painting is not just a representation of the night sky; it is a symbol of Van Gogh's inner world, a reflection of his emotional and mental state.Another noteworthy painting is Edvard Munch's "The Starry Night Over the Rhone." This work, painted in 1890, depicts a serene night scene with a star-filled sky and a calm river. Munch's use of soft, muted colors creates asense of tranquility and peace, in stark contrast to the more dynamic and emotional representation of the night skyin Van Gogh's work. This painting is a testament to Munch's ability to evoke different emotions and atmospheres through his paintings.More recently, painters like Jackson Pollock have experimented with new techniques to depict the starry skies. His "Convergence" series, for instance, features abstract paintings that use splatter and drip techniques to create a sense of the universe's vastness and infinity. These paintings are not rooted in traditional representational art, but they still evoke a sense of awe and wonder in the viewer.The starry sky paintings of Western art are not just visual treats; they are also profound explorations of the human mind and spirit. These paintings allow us to gaze at the night sky and feel a sense of connection to the universe, a reminder of our smallness within the vast cosmos, and a celebration of the beauty and mystery thatlies beyond our immediate grasp.**星空在西方艺术中的魅力**西方艺术在描绘星空方面有着丰富的历史,这些描绘夜晚奇观的画作赢得了无数观众的喜爱。
介绍宇宙英语作文

介绍宇宙英语作文The universe has always been a subject of fascination and curiosity for humanity. As we look up at the night sky, weare met with a myriad of stars, galaxies, and celestialbodies that spark our imagination and inspire us to explorethe unknown. In this essay, we will delve into the wonders of the universe, discussing its vastness, the mysteries it holds, and the impact it has on our understanding of existence.The Scale of the UniverseThe universe is incomprehensibly large, with observable dimensions spanning over 93 billion light-years. It is filled with countless stars, each potentially hosting their own planets and moons. The sheer scale of the cosmos is humbling, reminding us of our place in the grand scheme of things.Galaxies and StarsWithin the universe, galaxies are the cities of stars, with each galaxy containing billions of stars. Our own star, the Sun, is just one of the 200 billion stars in the Milky Way galaxy. Each star has its own life cycle, from formation to eventual death, either as a supernova or a slow-burning white dwarf.The Mystery of Dark Matter and Dark EnergyDespite the vastness of the universe, there is much we do not understand. Dark matter and dark energy are two such enigmas. Dark matter, which is believed to make up about 27% of the universe, does not emit or absorb light, making it invisible to our telescopes. Dark energy, on the other hand, is a hypothetical form of energy that is thought to be responsible for the accelerated expansion of the universe.The Search for Life Beyond EarthOne of the most profound questions in astronomy is whether there is life elsewhere in the universe. With the discovery of exoplanets—planets outside our solar system—scientists are now able to explore the possibility of life in environments vastly different from our own.The Impact on HumanityThe study of the universe has not only expanded our knowledge but also influenced our culture, inspiring art, philosophy, and even religion. It has led to technological advancements, such as satellite communication and GPS, which are integral to our modern way of life.The Future of Space ExplorationAs we continue to explore the universe, we are developing new technologies and methods to further our understanding. Projects like the James Webb Space Telescope and the Mars rover missions are at the forefront of this exploration, promising to reveal more about the origins of the universeand the potential for life on other planets.ConclusionThe universe is a vast and complex tapestry of celestial bodies and phenomena that continue to captivate our minds. As we continue to explore and learn, we gain a deeper appreciation for the cosmos and our place within it. The universe stands as a testament to the endless possibilities that exist beyond our own world, inviting us to dream, explore, and discover.。
介绍梵高星空英语作文小学

介绍梵高星空英语作文小学In the vast and mysterious world of art, there are few paintings that capture the imagination of both children and adults alike as Vincent van Gogh's "Starry Night." This masterpiece, which depicts a night sky filled with swirling clouds and blazing stars, is not just a representation of the heavens above; it's a gateway to a world of wonder and creativity.The first thing that catches the eye when looking at "Starry Night" is the vivid use of color. Van Gogh's brushstrokes are thick and bold, painting the sky in a blend of blues, yellows, and whites that are both realistic and surreal. The stars twinkle like diamonds scattered across a velvet blanket, while the moon shines brightly in the distance. The swirling clouds above add a sense of movement and dynamism to the composition, making the viewer feel as if they are looking into a living, breathing universe.But what makes this painting especially appealing to children is the sense of adventure and exploration it invokes. To a child's imagination, the night sky is a vastand unknown territory filled with mysterious creatures and exciting adventures. Van Gogh's painting allows them to escape into this imaginary world, where they can beanything they want to be: an astronaut exploring the cosmos, a wizard casting spells on the stars, or a dragon flying through the clouds.The lesson of creativity is also an important one for children. Van Gogh's use of bold brushstrokes and vivid colors encourages them to express themselves freely through art. He demonstrates that there are no rules when it comesto creating art, and that the most important thing is to express one's feelings and ideas. This message isinvaluable for young minds, who are constantly learning and growing.In addition to its visual appeal, "Starry Night" also has a deeper emotional impact on children. The painting evokes a sense of awe and wonder that can inspire childrento dream big and aim for the stars. It reminds them thatthe universe is vast and limitless, and that they have the potential to achieve anything they set their minds to.Moreover, "Starry Night" teaches children about the importance of perseverance. Van Gogh faced many challenges in his life, including mental illness and financial struggles, but he never gave up on his passion for art. His dedication and perseverance are reflected in his paintings, including "Starry Night," which he created while in a mental asylum. His story鼓励孩子们,无论遇到什么困难,都要坚持自己的梦想和热情,因为只有这样,他们才能创造出属于自己的辉煌。
梵高的星空对其进行美学标准英语作文

梵高的星空对其进行美学标准英语作文Van Gogh's Starry Night: A Whirlwind of BeautyHave you ever looked up at the night sky and got lost in all the twinkling stars? That's how I feel when I gaze at Van Gogh's famous painting "The Starry Night." It's like being sucked into a magical universe where the stars swirl and dance across the canvas. Let me tell you all about this awesome masterpiece!First off, the colors in this painting are simply out of this world! Van Gogh uses bright shades of yellow, blue, and green that make the whole scene vibrate with energy. The sky is a swirling vortex of yellows, blues, and greens all melting together. It's like the heavens opened up and spilled their cosmic paint across the night. Rad, right?Then there are the stars themselves. They're not just plain white dots - oh no! Van Gogh made them these deliciously thick globs of yellow and white paint that look like they're shimmering and pulsing. Some stars have shooting streams of paint radiating out, as if they're blazing comets racing across the sky. My favorite ones are the big whirly spiral stars that seem to be twisting and turning like cosmic ballerinas dancing among the heavens. Mind-blowing!Underneath this stellar swirl-fest is a peaceful little village along a winding river. The houses, trees, and church almost seem to glow under the magical night sky. The church steeple even looks like it's pointing up at the stars in amazement. I can imagine all the villagers stopping to gaze up at this crazy night canvas in wonder.To me, the best part is how Van Gogh made everything look like it's moving and alive, even though it's just a still painting. Those boomerang-shaped hills in the foreground remind me of waves rolling across the landscape. The trees almost seem to be shaking their branches in the celestial winds. And that swirling, spinning night sky makes my head go around and around just looking at it. It's like the whole world is whirling in a cosmic dance!I think Van Gogh created such an incredible, imaginative scene because he was able to see the night sky in a very special way. Most of us look up and just see stars studded across the darkness. But in Van Gogh's mind, the night became this exhilarating exhibition of light and motion - the stars weren't just twinkling, they were spinning in an intergalactic dance party! That's the sign of a true artist, being able to transform the ordinary into the extraordinary.Some people say Van Gogh was a tortured soul struggling with mental illness. But when I look at a masterpiece like Starry Night, I don't see the torment and anguish. I see the wonderment of a kid watching a kaleidoscope materialize in the heavens above. I see the awestruck vision of someone experiencing the miraculous beauty of nature in its purest, most rapturous form.In the end, that's what Starry Night means to me. It's a celebration of the sublime magic of the cosmos. It's a delirious dream of a night sky where up is down, stars blur into streaks of fire, and everything in the heavens dervishes in a whirling choreography of light. Thanks to Van Gogh's visionary genius, a ordinary starry night has been transformed into an exuberant, ecstatic ode to the glories and mysteries of the universe!。
小学上册第十二次英语第二单元全练全测

小学上册英语第二单元全练全测英语试题一、综合题(本题有100小题,每小题1分,共100分.每小题不选、错误,均不给分)1.I have a _____ of bubble gum. (piece)2.Batteries convert chemical energy into ______ energy.3.What do we call the natural habitat of an organism?A. EcosystemB. EnvironmentC. NicheD. CommunityC4. A _______ is a negatively charged particle found in an atom.5.I enjoy playing ______ at home.6.I enjoy making ________ (饼干) during the holidays.7. a _______ (红色的) dress to the party. She wrot8.What do you call the ability to see things clearly?A. VisionB. SightC. PerceptionD. ClarityA9.We have a ______ (有趣的) science experiment to do.10.I like to play with my friends in the ______ (公园). We have a lot of ______ (乐趣).11.My favorite animal is a _______ (我最喜欢的动物是_______).12.The panda eats _____ bamboo. (lots of)13.What is the longest bone in the human body?A. HumerusB. FemurC. TibiaD. RadiusB14.The ________ loves to hop around the garden.15.The main gas that contributes to global warming is __________.16.The rabbit's fur is _______ (柔软).17.What is the name of the fairy tale character who lost her glass slipper?A. Snow WhiteB. CinderellaC. RapunzelD. Sleeping Beauty18.What do we call the act of conserving resources?A. SustainabilityB. PreservationC. ConservationD. RecyclingC19.The parrot can mimic _______ (人类的声音).20.An electric current flows in a _______.21.What is the capital of Russia?A. MoscowB. St. PetersburgC. KyivD. MinskA22.The rabbit's favorite food is ________________ (胡萝卜).23. A flamingo stands on one leg to ______ (休息).24.What do you call the process of planting seeds?A. GrowingB. HarvestingC. SowingD. WateringC25.The first person to discover America was ______ (哥伦布).26.We like to ___ (play/sing) songs.27.Which vegetable is known for its green color and is often used in salads?A. PotatoB. LettuceC. CarrotD. OnionB28.The _____ (grapevine) produces delicious fruit.29.What is the capital city of the Bahamas?A. NassauB. FreeportC. Marsh HarbourD. George TownA30.What do we call the effect of the Earth's rotation on weather patterns?A. Coriolis EffectB. Trade WindsC. Jet StreamD. Ocean Currents31.The bat hangs _______ upside down.32. A reaction that occurs in the presence of heat is called a ______ reaction.33.Most plants perform ______ (光合作用) during the day.34.Planting trees can combat __________ (全球变暖).35.The _______ (Fall of the Berlin Wall) symbolized the end of the Cold War.36.My pet turtle likes to hide in its ______ (壳).37.Many plants change color in ______ (秋天).38.What do we call a person who studies the structure and function of proteins?A. BiochemistB. Molecular BiologistC. GeneticistD. MicrobiologistA39.Listen and number.听录音,给下列图片标号。
梵高星空介绍英文作文

梵高星空介绍英文作文英文:Vincent van Gogh's Starry Night is one of the most famous paintings in the world. The painting depicts a small village with a church and houses, surrounded by a swirling sky filled with stars. The painting is known for its bold colors and thick brushstrokes, which give the painting a sense of movement and energy.I have always been fascinated by this painting, and I think it's because of the way van Gogh captures the night sky. The swirling patterns and bright colors make the painting feel alive and dynamic. It's almost as if the sky is dancing above the village.One of my favorite things about Starry Night is the way van Gogh uses light. The stars and the moon are bright and vibrant against the dark sky, and the light from thevillage below casts a warm glow on the surroundinglandscape. It's a beautiful contrast between light and dark, and it makes the painting feel both peaceful and lively.Overall, I think Starry Night is a masterpiece of modern art. It's a painting that captures the beauty and energy of the night sky in a way that no other painting has ever done before.中文:梵高的《星夜》是世界上最著名的画作之一。
梵高星空的英文作文

梵高星空的英文作文英文:Vincent van Gogh's "Starry Night" is one of the most famous paintings in the world. The painting depicts a beautiful night sky with swirling clouds and bright stars. The painting has captured the imagination of people around the world and has become a symbol of the beauty of nature.When I first saw the painting, I was struck by thevivid colors and the sense of movement in the sky. The painting seems to come alive and I feel as if I am standing in the middle of the scene. The swirls of the clouds and stars create a sense of movement and energy that is both calming and exciting.What I love most about the painting is the way that it captures the beauty of the night sky. The stars are bright and shining, and the colors of the sky seem to glow with an otherworldly light. The painting reminds me of the wonderand awe that I feel when I look up at the stars on a clear night.Overall, "Starry Night" is a masterpiece that captures the beauty and wonder of nature. It is a painting that will continue to inspire people for generations to come.中文:梵高的《星夜》是世界上最著名的绘画之一。
萨比恩度数象征

Aries 白羊座1 A woman has risen from the water; a seal has emerged also, and is embracing her.一位女子从水面升起,一只海豹也随之出现,与她相拥。
2 A comedian is entertaining a group of friends.喜剧演员正款待他的一群朋友。
3 A cameo shows the profile of a man that suggests the outline of his country.贝壳浮雕上一个男子的侧脸令人想起他的祖国的样子。
4 Two lovers are strolling through a secluded walk.恋人正漫步着穿过一条幽谧的小径。
5 A white triangle is seen; it has golden wings.白色三角有着金色的边(精灵拥有希望之翼)。
6 A black square stands firmly, illumined red on one side.黑色的方块稳稳的伫立,其中的一面被照得通红。
7 A man comes forward flushed with the successful and simultaneous expression of his talents in two separate realms.男子自满于同时在两个独立领域里表现出的天赋。
8 A large old-fashioned woman's hat is revealed with streamers flying in a stiff breeze from the east.东边的和风吹起,一顶很大的旧式女帽下直直的飘带迎风飞舞。
9 A seer gazes intently into a crystal ball before him.先知专注地凝视着他面前的水晶球。
最终版星月夜中英文

Starry, starry nightPaint your palette blue and grayLook out on the summer’s dayWith eyes that know the darkness in my soulShadows on the hillsSketch the trees and the daffodilsCatch the breeze and the winter chillsIn colors on the snowy linen land星空,星夜画你的调色板蓝色和灰色看看夏天的一天用眼睛知道我的灵魂的黑暗阴影在山上草绘树和水仙抓住微风和冬季寒战在多雪的亚麻土地的颜色Now I think I know what you tried to say to meHow you suffered for your sanityHow you tried to set them freeThey would not listenThey’re not listening stillPerhaps they never will现在我想我知道你试图对我说什么你如何遭受你的理智你如何尝试将其设置为免费的他们不会听他们不是静静地听van Gogh(March 30,1853-July 29,1890)is generally considered the greatest Dutch painter after Rembrandt, though he had little success during his lifetime . Van Gogh produced all of his work(some 900 paintings and 1100 drawings)during a period of only 10 years before he succumbed to mental illness(possibly bipolar disorder)and committed suicide . His fame grew rapidly after his death especially following a showing of 71 of van Gogh’s paintings in Paris on March 17,1901(111903年3月30日至1890年7月29日)通常被认为是伦勃朗后最伟大的荷兰画家,虽然他在他的一生中没有成功。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
a r X i v :a s t r o -p h /0604012v 1 2 A p r 2006Astronomy &Astrophysics manuscript no.February 5,2008(DOI:will be inserted by hand later)White dwarf stars in D dimensionsP.H.ChavanisLaboratoire de Physique Th´e orique,Universit´e Paul Sabatier,118route de Narbonne 31062Toulouse,France e-mail:chavanis@irsamc.ups-tlse.frTo be included laterAbstract.We determine the mass-radius relation of relativistic white dwarf stars (a self-gravitating degenerate Fermi gas at T =0)in a D -dimensional universe and study the influence of the dimension of space on the laws of physics when we combine quantum mechanics,special relativity and gravity.We exhibit characteristic dimensions D =1,D =2,D =3,D =117),D =4,D =2(1+√1In this work,it is assumed that the system is completelydegenerate.The case of a partially degenerate self-gravitating Fermi gas at arbitrary temperature has been discussed more re-cently by Hertel &Thirring (1971)and Chavanis (2002a)in the context of statistical mechanics.They describe the phase tran-sition,below a critical temperature T c ,from a gaseous configu-ration to a condensed state (with a degenerate core surrounded by a halo).This provides a simple physical mechanism showing how the system can reach highly degenerate configurations as a result of gravitational collapse.structure is equivalent to a polytrope of index n =3/2so that the mass-radius relation of classical white dwarf stars behaves like MR 3∼1(Chandrasekhar 1931a).The next step was made by Chandrasekhar,aged only nine-teen,who was accepted by the University of Cambridge to work with Fowler.In the boat that took him from Madras to Southampton (Wali 1991),Chandrasekhar un-derstands that relativistic effects are important in mas-sive white dwarf stars and that Einstein kinematic must be introduced in the problem.In his first treatment (Chandrasekhar 1931b),he considers the ultra-relativistic limit and shows that the resulting structure is equiva-lent to a polytrope of index n =3.Applying the theory of polytropic gas spheres (Emden 1907),this leads to a unique value of the mass that he interprets as a limit-ing mass,nowdays called the Chandrasekhar limit.The complete mass-radius relation of relativistic white dwarf stars was given later (Chandrasekhar 1935)and departs from Fowler’s sequence as we approach the limiting mass.At this critical mass,the radius of the configuration van-ishes.Above the critical mass,the equation of state of the relativistic degenerate Fermi gas of electrons is not able to balance gravitational forces and,when considering the final evolution of such a star,Chandrasekhar (1934)“left speculating on other possibility”.Chandrasekhar’s result was severly criticized by Eddington (1935)who viewed this result as a reductio ad absurdum of the relativistic formula and considered the combination of special relativity and non-relativistic quantum theory as an “unholly alliance”.2P.H.Chavanis:White dwarf stars in D dimensionsBecause of the querelle with Eddington,it took some time to realize the physical implication of Chandrasekhar’s re-sults.However,progressively,his early investigations on white dwarf stars were extended in general relativity to the case of neutron stars(Oppenheimer&Volkoff1939)and finally led to the concept of“black holes”,a term coined by Wheeler in1967.Without the dispute with Eddington, this ultimate stage of matter could have been predicted much earlier from the discovery of Chandrasekhar(see Zakharov2002).Although these historic elements are well-known,it is less well-known that the concept of a maximum mass for relativistic white dwarf stars had been introduced earlier by Anderson(1929)and Stoner(1930).These studies are mentioned in the early works of Chandrasekhar but they have been progressively forgotten and are rarely quoted in classical textbooks of astrophysics.These authors in-vestigated the equation of state of a relativistic degener-ate Fermi gas and predicted an upper limit for the mass of white dwarf stars.Stoner(1930)uses a uniform mass density to model the star while Chandrasekhar(1931b) considers a more realistic n=3polytrope.However,as noted in Chandrasekhar(1931b),the value of the limiting mass found by Stoner with his simplified model is rela-tively close to that obtained with the improved treatment. Interestingly,Nauenberg(1972)introduced long after a simplified treatment of relativistic white dwarf stars in order to obtain an analytical approximation of the mass-radius relation.It turns out that this model,which gives a very good agreement with Chandrasekhar’s numerical results(up to some normalization factors),is equivalent to that introduced by Stoner.Leaving aside these interesting historical remarks,the result of Chandrasekhar(1931b)concerning the existence of a limiting mass is very profound because this mass can be expressed in terms of fundamental constants,similarly to the Bohr radius of the hydrogen atom(Chandrasekhar 1984).Hence,the mass of stars is determined typically by the following combinationhc H2≃29.2M⊙(1) where G is the constant of gravity,h the Planck constant, c the velocity of light and H the mass of the hydrogen atom.This formula results from the combination of quan-tum mechanics(h),special relativity(c)and gravity(G). Since dimensional analysis plays a fundamental role in physics,it is of interest to investigate how the preced-ing results depend on the dimension of space D of the universe.In our previous investigations(Chavanis&Sire 2004,Chavanis2004),we considered the case of classical white dwarf stars in D dimensions and found that they be-come unstable in a space of dimension D≥4.This means that quantum mechanics cannot stabilize matter against gravitational collapse in D≥4contrary to what happens in D=3(Fowler1926,Chavanis2002a).Interestingly, this is similar to a result found by Ehrenfest(1917)at the atomic level for Coulomb forces in Bohr’s model.The object of this paper is to extend these results to the case of relativisitic white dwarf stars and exhibit particular di-mensions of space which play a special role in the problem when we combine Newtonian gravity,quantum mechanics and special relativity.At the present stage,we do not aim at any physical application or interpretation of these re-sults.Our approach is essentially motivated by curiosity. We shall see,however,that the problem is very rich and interesting in its own right.It shows to which extent the dimension D=3of our universe is particular.A connec-tion with other works investigating the role played by the dimension of space on the laws of physics is made in the Conclusion.2.The equation of stateIn the completely degenerate limit,the electrons have mo-menta less than a threshold value p0(Fermi momentum) and their distribution function is f=2/h D where h is the Planck constant.There can only be two electrons in a phase space element of size h D on account of the Pauli exclusion principle.Therefore,the number of electrons per unit volume isn= fd p=2S D Dh D p D0.(2) The mean kinetic energy per electron is given byκ=1nh D p00ǫ(p)p D−1dp,(3) whereǫ(p)is the energy of an electron with impulse p.In relativistic mechanics,ǫ=mc2 1+p2D fp dǫDh Dp00p D dǫDmh D p00p D+1m2c2)1/2dp.(6) Finally,the mass density isρ=nµH(7)where H is the mass of the proton andµthe molecular weight.If we consider a pure gas of fermions(like,e.g., massive neutrinos in dark matter models),we just have to replaceµH by their mass m.Introducing the notation x=p0/mc,we can write the density of state parametrically as followsP=A2f(x),ρ=Bx D,(8)P.H.Chavanis:White dwarf stars in D dimensions 3where A 2=S D m D +1c D +2Dh D,(9)f (x )=8xt D +1D +2x D +2(x ≪1)(11)f (x )≃8dr=−GM (r )r D −1d ρdPr D −1d dr=−S D GB 2S D G1/212,y 20=1+x 20.(18)Note that the scale of length a is independent ony 0for D = 1.Substituting these transformations in Eq.(16),we obtain the D -dimensional generalization of Chandrasekhar’s differential equation1dη ηD −1dφy 20 D/2,(19)with the boundary conditions φ(0)=1,φ′(0)=0.(20)The radius R of the star is such that ρ(R )=0.This yieldsφ(η1)=1(y 20−1)D/2φ2−1S D GD/212(3−D )ηD −1dφD,(25)with K 1=12S D2m (µH )D +22.(27)Polytropic stars are described by the Lane-Emden equa-tion (Emden 1907).This can be recovered as a limit of the Chandrasekhar equation.For x ≪1,we have y 0≃1+12(x 20−θ).Setting ξ=√ξD −1ddξ=−θD/2,(28)θ(0)=x 20,θ′(0)=0.(29)Note that the condition at the origin is θ(0)=x 20in-stead of θ(0)=1as in the ordinary Lane-Emden equa-tion.However,using the homology theorem for polytropicspheres (Chandrasekhar 1942),we can easily relate θto θD/2,the solution of the Lane-Emden equation with index n 3/2=D/2and condition at the origin θ(0)=1.The structure and stability of polytropic spheres in various dimensions of space has been studied by Chavanis &Sire (2004).This study exhibits two important indices:n 3=DD −2.(30)4P.H.Chavanis:White dwarf stars in D dimensions−20246ln(α)246810ηD=5D=4.5D=4D=3.5D=3D=1n 3/2=D/2Fig.1.Relation between the mass (ordinate)and the cen-tral density (abscissa)of box-confined polytropes withindex n 3/2=D/2in an appropriate system of coordi-nates (see Chavanis &Sire 2004and Sec.8.1for details).Complete polytropes correspond to the terminal point in the series of equilibria.The series becomes dynamically unstable with respect to the Euler-Poisson system (saddle point of the energy functional)after the turning point of mass ηwhich appears for D =4.Thus,classical white dwarf stars become unstable for D >4.1234M/M 01234R /R 0D=3D=1D=4D=2Fig.2.Mass-Radius relation for classical white dwarf stars in different dimensions of space.The radius is in-dependent on mass in D =2and the mass is independent on radius in D =4.The dimensional factors M 0and R 0are defined in Sec.6According to this study,a classical white dwarf star is self-confined (complete)if n 3/2<n 5,i.e.D <2(1+√DR 4−D =K 1(D +2)DD/2,(31)where we have definedωD/2=−ξD +2DR4−D=12S 2D2/Dh 2DωD −22)itincreases with the radius (see Fig.2).For D =4the mass is independent on the radius and given in terms of fundamental constants by M =ω2m 2G 2(µH )3≃0.0143958...h 416π6h 4m 2G 2(µH )3.(35)For D =2,the radius is independent on mass and given in terms of fundamental constants byR =ξ12πh(Gm )1/2µH.(36)Furthermore,for D =2,the Lane-Emden equation (28)with index n 3/2=1can be solved analytically,yielding θ1=J 0(ξ)and ξ1=α0,1where α0,1=2.404826...is the first zero of Bessel function J 0.We obtain a density profileρ(r )=ρ0J 0(ξ1r/R ),(37)where the central density is related to the total mass by M =−ρ0Gm (µH )2ξ1θ′1=0.0993492...ρ0h 2P.H.Chavanis:White dwarf stars in D dimensions 55.The ultra-relativistic limitIn the ultra-relativistic limit x ≫1,we find that the equa-tion of state takes the form P =K 2ρ1+1D +1DDhcD.(40)Therefore,an ultra-relativistic white dwarf star is equiva-lent to a polytrope of index n ′3=D.(41)This also directly results from the Chandrasekhar equa-tion.For x ≫1,it reduces to1dξξD −1dθ2(3+√DR 3−D =K 2(D +1)DD,(44)where we have definedωD =−ξD +1DR 3−D =DG (µH )D +1DD.(46)For 3<D <117),the mass M increases as the radius R increaseswhile for 1<D <3it decreases with the radius (see Fig.4).For D =1,the radius is independent on mass and given in terms of fundamental constants byR =ξ12S 1 hc µH =0.555360... hc µH .(47)Furthermore,for D =1,the Lane-Emden equation (42)with index n ′3=1can be solved analytically,yielding θ1=cos(ξ)and ξ1=π/2.We obtain a density profile ρ(r )=ρ0cos(ξ1r/R ),(48)−11357ln(α)0123ηn’3=DD=2D=3D=3.3D=4Fig.3.Relation between the mass (ordinate)and the cen-tral density (abscissa)of box-confined polytropes with in-dex n ′3=D in an appropriate system of coordinates (see Chavanis &Sire 2004and Sec.8.1for details).Complete polytropes correspond to the terminal point in the series of equilibria.The series becomes dynamically unstable with respect to the Euler-Poisson system (saddle point of the energy functional)after the turning point of mass ηwhich appears for D =3.Thus,ultra-relativistic white dwarf stars become unstable for D >3.01234M/M 0R /R 0D=2D=3D=1Fig.4.Mass-Radius relation for ultra-relativistic white dwarf stars in different dimensions of space.The radius is independent on mass in D =1and the mass is indepen-dent on radius in D =3(Chandrasekhar’s mass).where the central density is related to the total mass by M =ρ02hcµH.(49)6P.H.Chavanis:White dwarf stars in D dimensionsFor D =3,the mass is independent on radius and given in terms of fundamental constants byM =3G3/21G3/21M 0=yDR 0=12η1,(52)where we have definedM 0=S D8A 2B D −1,R 0=8A 2B,(53)andΩ(y 0)=−ηD −1dφ2S 2D1/2h D/22c D −22S 2DD −22c(4−D )Dm(D −3)D(µH )D −1.(56)We can now obtain the mass-radius curve M −R by thefollowing procedure.We fix avalue of the parameter y 0and solve the differential equation (19)with initial con-dition (20)until the point η=η1,determined by Eq.(21),at which the density vanishes.The radius and the mass of the corresponding configuration are then given by Eqs.(52)a-b.By varying y 0,we can obtain the full curve R (y 0)−M (y 0)parameterized by the value of the central density ρ0given by Eq.(23).To solve the differ-ential equation (19),we need the behavior of φat the origin.Expanding φ(η)in Taylor series and substituting this expansion in Eq.(19)we obtain for η→0:φ=1−q D8(D +2)q 2(D −1)η4+...(57)where q 2=1−1M 0D −2R 04−D=1DD/2.(59)In the ultra-relativistic limit,using Eq.(46),we getMDRDD.(60)In Figs.5-12,we plot the mass-radius relation of relativis-tic white dwarf stars (full line)for different dimensions of space.The asymptotic relations (59)and (60)valid in the classical (C)and ultra-relativistic (R)limits are also shown for comparison (dashed line)together with the an-alytical approximation (dotted line)derived in Sec.7.P.H.Chavanis:White dwarf stars in D dimensions75101520M/M 000.511.52R /R 0D=1.5(C)(R)Fig.6.Mass-radius relation in D =1.5.The configura-tions are always stable and there exists a maximum radius for partially relativistic distributions.M/M 00.511.52R /R 0Fig.7.Mass-radius relation in D = 2.The radius de-creases with the mass.The configurations are always sta-ble and there exists a limiting radius achieved in the clas-sical limit for M →0.7.Analytical approximation of the mass-radius relationWe present here an analytical approximation of the mass-radius relation in various dimensions of space based on the treatment by Nauenberg (1972)in D =3.This treat-ment amounts to considering that the density is uniform in the star and the mass-radius relation is obtained by minimizing the energy functional with respect to the ra-dius (or the density)at fixed mass.As mentioned in the Introduction,this is similar to the simplified model of rel-ativistic white dwarf stars made by Stoner (1930)before Chandrasekhar’s treatment.0246810M/M 0123R /R 0D=2.5(C)(R)Fig.8.Mass-radius relation in D =2.5.The radius de-creases with the mass.The configurations are always sta-ble.00.51 1.52M/M 0123456R /R 0(R)(C)D=3Fig.9.Mass-radius relation in D = 3.The radius de-creases with the mass.The configurations are always sta-ble and there exists a limiting mass (Chandrasekhar’s mass)achieved in the ultra-relativistic limit for R =0.For M >M Chandra ,quantum mechanics cannot arrest gravitational collapse.Following Nauenberg (1972),we approximate the ki-netic energy K by the form K =Nmc21+p 22Note that Stoner (1930)uses the exact expression (3)of the kinetic energy in his treatment.This leads to more com-plicated expressions with,however,qualitatively similar con-clusions.Since the models are based on the (illicit)approxi-8P.H.Chavanis:White dwarf stars in D dimensions00.20.40.60.8M/M 05101520R /R 0D=3.3(C)(R)Fig.10.Mass-radius relation in D =3.3.There ex-ists a limiting mass for partially relativistic distributions.Classical configurations are stable and the series of rela-tivistic equilibria becomes unstable after the turning point of mass.Thus,highly relativistic configurations in D >3cannot be in hydrostatic equilibrium.assume that it is determined by an appropriate average value of the density by the relation ρ=2S DS D R D,(63)where ζis a dimensionless parameter.We also write thepotential energy in the form W =−νR D −2,(64)where νis another dimensionless parameter.By writingEq.(64),we have assumed that D =2but we shall see that the following results pass to the limit for D →2.We introduce two dimensionless variables n and r and two fixed constants M ∗=N ∗µH and R ∗such that M =nM ∗,R =rR ∗.(65)We determine M ∗and R ∗by the requirement that the relativity parameter x =p/mc have the form x =n 1/DD −2mc 2N ∗n 2mation that the density is homogeneous,the results cannot be expected to be more than qualitatively correct.Therefore,the approximation (61)made by Nauenberg for the kinetic energy is sufficient for the purposes of this simplified approach.00.51 1.52M/M 010203040R /R 0D=3.8(C)(R)Fig.11.Mass-radius relation in D =3.8.For D >117)the ultra-relativistic configurations (equiva-lent to polytropes of index n ′3=D )are not self-confined anymore and a spiral develops in the mass-radius relation.This is somehow similar to the classical spiral occurring in the (E,β)plane in the thermodynamics of self-gravitating systems (see,e.g.,Katz 1978)and to the spiral occurring in the (M,R )plane in the general relativistic treatment of neutron stars (see,e.g.,Meltzer &Thorne 1966,Chavanis 2002c).The series of equilibria becomes unstable at the first turning point of mass and new modes of instabil-ity occur at the secondary turning points (see Shapiro &Teukolsky 1983).This yieldsR ∗= DζG 1/2m D −12µH,(68)M ∗=12S 2DD −22cD (4−D )mD (D −3)(µH )D −1.(69)Comparing with Eqs.(55)and (56),we find thatM ∗=12M 0,R ∗= ζD −2.(71)We shall consider the energy as a function of the radius R ,with the mass M fixed.Thus,the mass-radius relation will be obtained by minimizing the energy E versus x with fixed n .Writing ∂E/∂x =0,we obtain the equations n =xD(1+x 2)D/4,(72)x =n 1/DP.H.Chavanis:White dwarf stars in D dimensions90123M/M 010203040R /R 0D=4(R)(C)Fig.12.Mass-radius relation in D =4.There exists a lim-iting mass achieved in the classical limit for R →+∞.In fact,for D ≥4,all the configurations (classical and rela-tivistic)are unstable.Quantum mechanics cannot balance gravitational attraction even in the classical limit.defining the mass-radius relation in parametric form in the framework of the simplified model.Note that x D rep-resents the value of the density in units of M ∗/R D∗,i.e.ρ=x D M ∗/R D∗.Therefore,Eq.(72)can be viewed as the relation between the mass and the density.Eliminating the relativity parameter x (or density)between Eqs.(72)and (73),we explicitly obtain n =r 31−r 4(D =1)(74)n =1−r 4n 1/3(D =3)(76)r =n 3/41−n(D =4)(77)In order to compare with the exact results,we need to determine the values of the constants ζand ν.This can be done by considering the limiting forms of Eqs.(72)and (73).In the classical limit x ≪1,we obtain nD −2Dr 3−D =1.(79)Comparing with Eqs.(59)and (60),we find that ζ=1ωD −1D,ν=1DD/2DD.(80)The analytical approximations of the mass-radius relation (74)-(77)are plotted in dotted lines in Figs.5,7,9;they give a fair agreement with the exact results (full line).Of course,they cannot reproduce the spiral in D =4which requires the resolution of the full differential equation.Finally,we address the stability of the configurations within this simplified analytical model.If we view a white dwarf star as a gas of electrons at statistical equilibrium at temperature T =0,stable configurations are those that minimize the free energy F =E −T S at fixed mass,where E is the total energy (kinetic +potential)and S is the Fermi-Dirac entropy.Now,for a completely degener-ate gas,S =0.Therefore,stable configurations minimize the energy E at fixed mass.As we have seen previously the first order variations E ′(x )=0determine the Mass-Radius relation.Then,the configurations is stable if the energy is minimum,i.e.E ′′(x )>0.Now,we have E ′′=N ∗mc 2nD −31/2.(82)We now show that the onset of instability precisely corre-sponds to the turning point of mass,i.e.to the point where the mass is maximum in the series of equilibria M (R ).In terms of reduced variables,this corresponds to dn/dr =0,or equivalently n ′(x )=0.Taking the logarithmic deriva-tive of Eq.(72),we have dn2(4−D )dx2x10P.H.Chavanis:White dwarf stars in D dimensionsSire 2004,2005a,Chavanis 2006a).Incomplete polytropes are confined by the box (ρ(R )=0)while complete poly-tropes are such that their density vanishes at R ∗≤R .Their nonlinear dynamical stability with respect to the Euler-Poisson system can be analyzed by plotting the se-ries of equilibria and using the Poincar´e turning point ar-gument.The series of equilibria is obtained by relating the parameterη=M K (n +1) nR(D −2)n −DK (n +1)1/2R.(85)There are different ways to read the η(α)relation.The pa-rameter ηis proportional to the mass while the parameterαdepends on the central density.Therefore,the η(α)rela-tion can be viewed as a relation M (ρ0)between the mass and the central density (for given R and K ).Alternatively,using a thermodynamical analogy (Chavanis 2006a),ηcan be seen as an effective inverse temperature.This analogy can be made more precise if we define a polytropic tem-perature Θ=K (1+n )n −1Mn −1R(D −2)n −D4ππGK 21/2R.(89)Incomplete polytropes confined by the box have α<ξ1=6.89685...while complete polytropes with R ∗≤R have η=ω3=2.01823595...and α=ξ1R/R ∗.Since the curve η(α)does not present turning points,the whole series of equilibria is nonlinearly dynamically stable.These stable equilibrium configurations exist only for η≤ηc =ω3.Forη=ηc,the configuration has infinite densityα→+∞and vanishing radius R∗=0,corresponding to a Dirac peak.In terms of the polytropic temperature(86),theparameterηcan be writtenη=ω3 Θc3(ω3)2/3,(91)where we emphasize that the critical temperatureΘc does not depend on the box radius.Now,for ultra-relativistic white dwarf stars,the constant K2is given in terms of fundamental constants by Eq.(40).In terms of the mass, the parameterηcan be writtenη=ω3MT,(94) where we have set k B=1.Like in Eq.(91),we note that it does not depend on the box radius.The parameterαis defined byα= 2πGρ04.(96) Alternatively,in terms of the mass,equilibrium configu-rations exist only forM≤M c=4TChavanis et al.(2004).In D=2,the series of equilibria of bacterial aggregates is identical to the caloric curve of isothermal self-gravitating systems in Fig.14but of course the parameters have another interpretation.In the biolog-ical context,ηtakes the formη=χλMχλ,(99)where M c is the maximum mass of bacterial populations. For M<M c there exists equilibrium configurations of bacterial colonies in a box(in an infinite domain the sys-tem evaporates,see Chavanis&Sire2005b).For M≥M c, the system collapses and forms a Dirac peak.Owing to these formal analogies,we see that the limiting mass M chandra of Chandrasekhar for relativistic white dwarf stars in D=3is similar to the limiting mass M c of bacte-rial populations in D=2which is itself similar to the limiting temperature T c of a bounded two-dimensional isothermal gas(Chavanis2006b).Of course,there is a crucial difference between the two systems because the Chandrasekhar mass is expressed in terms of fundamen-tal constants while the critical mass of bacterial popula-tions depends on non-universal parameters(diffusion co-efficient,mobility,...)which characterize a given bacterial population in the framework of the Keller-Segel model. These parameters change from one population to the other and they usually have to be adapted to the situation. However,the important point is that the critical mass is independent on the size of the box R and is intrinsic to the system under consideration(white dwarfs or bacterial colonies).8.4.Series of equilibria for partially relativistic whitedwarf starsIn the approach of Sec.8.1,we have assumed that the gas is always ultra-relativistic(polytrope of index3)and that for M<M chandra it is confined by the walls of an arti-ficial box.The more physical case of a partially relativis-tic gas that is self-confined(without box)can be treated by solving the Chandrasekhar equation(19).In Fig.15, we plot the mass M/M chandra as a function of the cen-tral densityρ0.This curve presents a behavior similar to that of Sec.8.1so that the above-mentioned analogies re-main valid in this more physical situation.Therefore,the Chandrasekhar limiting mass for relativistic white dwarf stars in D=3is really similar to the limiting mass of bacterial populations in D=2.−7−238131823ln(ρ/B)0.20.40.60.811.2M/M chandraD=3Fig.15.Series of equilibria for a partially relativistic white dwarf star.Equilibrium states exist only for M< M chandra.For M=M chandra,the density profile is a Dirac peak.9.Conclusion“Why is our universe three dimensional?Does the dimen-sion D=3play a special role among other space dimen-sions?”Several scientists have examined the role played by the dimension of space in determining the form of the laws of physics.This question goes back to Ptolemy who argues in his treatise On dimensionality that no more than three spatial dimensions are possible in Nature.In the18th century,Kant realizes the deep connection be-tween the inverse square law of gravitation and the ex-istence of three spatial dimensions.In the twentieth cen-tury,Ehrenfest(1917),in a paper called In what way does it become manifest in the fundamental laws of physics that space has three dimensions?argues that planetary orbits, atoms and molecules would be unstable in a space of di-mension D≥4.Other investigations on dimensionality are reviewed in the paper of Barrow(1983).We have found that the self-gravitating Fermi gas at T=0(a white dwarf star)possesses a rich structure as a function of the dimen-sion of space.We have exhibited several characteristic di-mensions D=1,D=2,D=3,D=117),D=4 and D=2(1+√。
