On irreducible four--manifolds
On area-stationary surfaces in certain neutral Kaehler 4-manifolds

In the next section we discuss neutral K¨ ahler metrics and the some of their properties. We also outline the construction of the neutral K¨ ahler structure on TN and the geometric structures induced on surfaces in TN. In the following section we derive the equations for area-stationary graphs in TN and show that holomorphic curves are area-stationary. We also show that lagrangian area-stationary graphs are totally null. In section 3 we look at rotationally symmetric graphs and determine all of these that area-stationary. In addition, we give a construction for surfaces on which the induced metric is degenerate at every point. In the final section, we look at the case of TS2 , which we identify with the space L of oriented affine lines in R3 . There we construct area-stationary tori that are neither holomorphic nor lagrangian, and investigate their geometric properties. This two-parameter family of surfaces are analogous to the catenoid in R3 , being the unique rotationally symmetric area-stationary surfaces in L. ¨hler Metric on TN 1. The Neutral Ka We begin with some general properties of a K¨ ahler surface (M, G, J, Ω). That is, M is a real 4-manifold endowed with the following structures. First, there is the metric G, which we do not insist be positive definite - it may also have neutral signature (+ + −−). In order to deal with both cases simultaneously we assume that the metric can be diagonalised pointwise to (1, 1, ǫ, ǫ), for ǫ = ±1. In addition, we have a complex structure J, which is a mapping J : Tp M → Tp M at each p ∈ M, which satisfies J2 = −Id and an integrability condition. Finally, there is a symplectic form Ω, which is a closed, non-degenerate 2-form. These structures are required to satisfy the compatibility conditions: G(J·, J·) = G(·, ·) G(·, ·) = Ω(J·, ·).
低维拓扑简介

考研论坛»数学»低维拓扑knight51发表于2005-7-28 08:34低维拓扑<P>下面说说低维拓扑的内容:低维拓扑是微分拓扑的一部分,主要研究3,4维流形与纽结理论。
又叫几何拓扑。
主要以代数拓扑与微分拓扑为工具。
它与微分几何和动力系统关系密切。
国外搞这个方向的也几乎都搞微分几何和动力系统。
我国这个方向北大最牛,美国是伯克利和普林斯顿最牛。
比起代数几何来,它比较好入门。
初学者只需要代数拓扑,微分拓扑,黎曼几何的知识就行了。
美国这方面比较牛,几乎每个搞基础数学研究的都会低维拓扑。
</P><DIV class=postcolor>纠正一下上面的错误,美国也不是每个搞基础数的都精通低维拓扑,而是懂一些低维拓扑的知识。
如果入门后还想更加深入了解它,那还需要读一些双曲几何和拓扑动力系统的书。
</DIV><!-- THE POST --><!-- THE POST --><DIV class=postcolor>下面介绍一下这方面的牛人:Bill Thurston studied at New College, Sarasota, Florida. He received his B.S. from there in 1967 and moved to the University of California at Berkeley to undertake research under Morris Hirsch's and Stephen Smale's supervision. He was awarded his doctorate in 1972 for a thesis entitled Foliations of 3-manifolds which are circle bundles. This work showed the existence of compact leaves in foliations of 3-dimensional manifolds.After completing his Ph.D., Thurston spent the academic year 1972-73 at the Institute for Advanced Study at Princeton. Then, in 1973, he was appointed an assistant professor of mathematics at Massachusetts Institute of Technology. In 1974 he was appointed professor of mathematics at Princeton University.Throughout this period Thurston worked on foliations. Lawson ([5]) sums up this work:-It is evident that Thurston's contributions to the field of foliations are of considerable depth. However, what sets them apart is their marvellous originality. This is also true of his subsequent work on Teichmüller space and the theory of 3-manifolds.In [8] Wall describes Thurston's contributions which led to him being awarded a Fields Medal in 1982. In fact the1982 Fields Medals were announced at a meeting of the General Assembly of the International Mathematical Union in Warsaw in early August 1982. They were not presented until the International Congress in Warsaw which could not be held in 1982 as scheduled and was delayed until the following year. Lectures on the work of Thurston which led to his receiving the Medal were made at the 1983 International Congress. Wall, giving that address, said:-Thurston has fantastic geometric insight and vision: his ideas have completely revolutionised the study of topology in 2 and 3 dimensions, and brought about a new and fruitful interplaybetween analysis, topology and geometry.Wall [8] goes on to describe Thurston's work in more detail:-The central new idea is that a very large class of closed 3-manifolds should carry a hyperbolic structure - be the quotient of hyperbolic space by a discrete group of isometries, or equivalently, carry a metric of constant negative curvature. Although this is a natural analogue of the situation for 2-manifolds, where such a result is given by Riemann's uniformisation theorem, it is much less plausible - even counter-intuitive - in the 3-dimensional situation.Kleinian groups, which are discrete isometry groups of hyperbolic 3-space, were first studied by Poincaré and a fundamental finiteness theorem was proved by Ahlfors. Thurston's work on Kleinian groups yielded many new results and established a well known conjecture. Sullivan describes this geometrical work in [6], giving the following summary:-Thurston's results are surprising and beautiful. The method is a new level of geometrical analysis - in the sense of powerful geometrical estimation on the one hand, and spatial visualisation and imagination on the other, which are truly remarkable.Thurston's work is summarised by Wall [8]:-Thurston's work has had an enormous influence on 3-dimensional topology. This area has a strong tradition of 'bare hands' techniques and relatively little interaction with other subjects. Direct arguments remain essential, but 3-dimensional topology has now firmly rejoined the main stream of mathematics.Thurston has received many honours in addition to the Fields Medal. He held a Alfred P Sloan Foundation Fellowship in 1974-75. In 1976 his work on foliations led to his being awarded the Oswald Veblen Geometry Prize of the American Mathematical Society. In 1979 he was awarded the Alan T Waterman Award, being the second mathematician to receive such an award (the first being Fefferman in 1976).</DIV><!-- THE POST -->第2个牛人:Michael Freedman entered the University of California at Berkeley in 1968 and continued his studies at Princeton University in 1969. He was awarded a doctorate by Princeton in 1973 for his doctoral dissertation entitled Codimension-Two Surgery. His thesis supervisor was William Browder.After graduating Freedman was appointed a lecturer in the Department of Mathematics at the University of California at Berkeley. He held this post from 1973 until 1975 when he became a member of the Institute for Advanced Study at Princeton. In 1976 he was appointed as assistant professor in the Department of Mathematics at the University of California at San Diego.Freedman was promoted to associate professor at San Diego in 1979. He spent the year 1980/81 at the Institute for Advanced Study at Princeton returning to the University of California at San Diego where he was promoted to professor on 1982. He holds this post in addition to the Charles Lee Powell Chair of Mathematics which he was appointed to in 1985.Freedman was awarded a Fields Medal in 1986 for his work on the Poincaré conjecture. The Poincaré conjecture, one of the famous problems of 20th-century mathematics, asserts that a simply connected closed 3-dimensional manifold is a 3-dimensional sphere. The higher dimensional Poincaréconjecture claims that any closed n-manifold which is homotopy equivalent to the n-sphere must be the n-sphere. When n = 3 this is equivalent to the Poincaré conjecture. Smale proved the higher dimensional Poincaré conjecture in 1961 for n at least 5. Freedman proved the conjecture for n = 4 in 1982 but the original conjecture remains open.Milnor, describing Freedman's work which led to the award of a Fields Medal at the International Congress of Mathematicians in Berkeley in 1986, said:-Michael Freedman has not only proved the Poincaré hypothesis for 4-dimensional topological manifolds, thus characterising the sphere S4, but has also given us classification theorems, easy to state and to use but difficult to prove, for much more general 4-manifolds. The simple nature of his results in the topological case must be contrasted with the extreme complications which are now known to occur in the study of differentiable and piecewise linear 4-manifolds. ... Freedman's 1982 proof of the 4-dimensional Poincaré hypothesis was an extraordinary tour de force. His methods were so sharp as to actually provide a complete classification of all compact simply connected topological 4-manifolds, yielding many previously unknown examples of such manifolds, and many previously unknown homeomorphisms between known manifolds.Freedman has received many honours for his work. He was California Scientist of the Year in 1984 and, in the same year, he was made a MacArthur Foundation Fellow and also was elected to the National Academy of Sciences. In 1985 he was elected to the American Academy of Arts and Science. In addition to being awarded the Fields Medal in 1986, he also received the Veblen Prize from the American Mathematical Society in that year. The citation for the Veblen Prize reads (see [3]):-After the discovery in the early 60s of a proof for the Poincaré conjecture and other properties of simply connected manifolds of dimension greater than four, one of the biggest open problems, besides the three dimensional Poincaré conjecture, was the classification of closed simply connected four manifolds. In his paper, The topology of four-dimensional manifolds, published in the Journal of Differential Geometry (1982), Freedman solved this problem, and in particular, the four-dimensional Poincaré conjecture. The major innovation was the solution of the simply connected surgery problem by proving a homotopy theoretic condition suggested by Casson for embedding a 2-handle, i.e. a thickened disc in a four manifold with boundary.Besides these results about closed simply connected four manifolds, Freedman also proved:(a) Any four manifold properly equivalent to R4 is homeomorphic to R4; a related result holds for S3 R.(b) There is a nonsmoothable closed four manifold.© The four-dimensional Hauptvermutung is false; i.e. there are four manifolds with inequivalent combinatorial triangulations.Finally, we note that the results of the above mentioned paper, together with Donaldson's work, produced the startling example of an exotic smoothing of R4.In his reply Freedman thanked his teachers (who he said included his students) and also gave some fascinating views on mathematics [3]:-My primary interest in geometry is for the light it sheds on the topology of manifolds. Here it seems important to be open to the entire spectrum of geometry, from formal to concrete. By spectrum, I mean the variety of ways in which we can think about mathematical structures. At one extreme the intuition for problems arises almost entirely from mental pictures. At the other extreme the geometric burden is shifted to symbolic and algebraic thinking. Of course this extreme is only a middle ground from the viewpoint of algebra, which is prepared to go much further in the direction of formal operations and abandon geometric intuition altogether.In the same reply Freedman also talks about the influence mathematics can have on the world and the way that mathematicians should express their ideas:-In the nineteenth century there was a movement, of which Steiner was a principal exponent, to keep geometry pure and ward off the depredations of algebra. Today I think we feel that much of the power of mathematics comes from combining insights from seemingly distant branches of the discipline. Mathematics is not so much a collection of different subjects as a way of thinking. As such, it may be applied to any branch of knowledge. I want to applaud the efforts now being made by mathematicians to publish ideas on education, energy, economics, defence, and world peace. Experience inside mathematics shows that it isn't necessary to be an old hand in an area to make a contribution. Outside mathematics the situation is less clear, but I cannot help feeling that there, too, it is a mistake to leave important issues entirely to experts.In June 1987 Freedman was presented with the National Medal of Science at the White House by President Ronald Reagan. The following year he received the Humboldt Award and, in 1994, he received the Guggenheim Fellowship Award.<DIV class=postcolor>介绍第3个牛人:Simon Donaldson's secondary school education was at Sevenoaks School in Kent which he attended from 1970 to 1975. He then entered Pembroke College, Cambridge where he studied until 1980, receiving his B.A. in 1979. One of his tutors at Cambridge described him as a very good student but certainly not the top student in his year. Apparently he would always come to his tutorials carrying a violin case.In 1980 Donaldson began postgraduate work at Worcester College, Oxford, first under Nigel Hitchen's supervision and later under Atiyah's supervision. Atiyah writes in [2]:-In 1982, when he was a second-year graduate student, Simon Donaldson proved a result that stunned the mathematical world.This result was published by Donaldson in a paper Self-dual connections and the topology of smooth 4-manifolds which appeared in the Bulletin of the American Mathematical Society in 1983. Atiyah continues his description of Donaldson's work [2]:-Together with the important work of Michael Freedman ..., Donaldson's result implied that there are "exotic" 4-spaces, i.e. 4-dimensional differentiable manifolds which are topologically but not differentiably equivalent to the standard Euclidean 4-space R4. What makes this result so surprising is that n = 4 is the only value for which such exotic n-spaces exist. These exotic 4-spaces have the remarkable property that (unlike R4) they contain compact sets which cannot be contained inside any differentiably embedded 3-sphere !After being awarded his doctorate from Oxford in 1983, Donaldson was appointed a Junior Research Fellow at All Souls College, Oxford. He spent the academic year 1983-84 at the Institute for Advanced Study at Princeton, After returning to Oxford he was appointed Wallis Professor of Mathematics in 1985, a position he continues to hold.Donaldson has received many honours for his work. He received the Junior Whitehead Prize from the London Mathematical Society in 1985. In the following year he was elected a Fellow of the Royal Society and, also in 1986, he received a Fields Medal at the International Congress at Berkeley. In 1991 Donaldson received the Sir William Hopkins Prize from the Cambridge Philosophical Society. Then, the following year, he received the Royal Medal from the Royal Society. He also received the Crafoord Prize from the Royal Swedish Academy of Sciences in 1994:-... for his fundamental investigations in four-dimensional geometry through application of instantons, in particular his discovery of new differential invariants ...Atiyah describes the contribution which led to Donaldson's award of a Fields Medal in [2]. He sums up Donaldson's contribution:-When Donaldson produced his first few results on 4-manifolds, the ideas were so new and foreign to geometers and topologists that they merely gazed in bewildered admiration.Slowly the message has gotten across and now Donaldson's ideas are beginning to be used by others in a variety of ways. ... Donaldson has opened up an entirely new area; unexpected and mysterious phenomena about the geometry of 4-dimensions have been discovered. Moreover the methods are new and extremely subtle, using difficult nonlinear partial differential equations. On the other hand, this theory is firmly in the mainstream of mathematics, having intimate links with the past, incorporating ideas from theoretical physics, and tying in beautifully with algebraic geometry.The article [3] is very interesting and provides both a collection of reminiscences by Donaldson on how he came to make his major discoveries while a graduate student at Oxford and also a survey of areas which he has worked on in recent years. Donaldson writes in [3] that nearly all his work has all come under the headings:-(1) Differential geometry of holomorphic vector bundles.(2) Applications of gauge theory to 4-manifold topology.and he relates his contribution to that of many others in the field.Donaldson's work in summed up by R Stern in [6]:-In 1982 Simon Donaldson began a rich geometrical journey that is leading us to an exciting conclusion to this century. He has created an entirely new and exciting area of research through which much of mathematics passes and which continues to yield mysterious and unexpected phenomena about the topology and geometry of smooth 4-manifolds</DIV><DIV class=postcolor>下面continue介绍第4个牛人:Robion Kirby。
Homologically Trivial Group Actions on 4-Manifolds

2
The Borel Fibering and Spectral Sequence
We follow Bredon [3], Chapter VII, or tom Dieck [5], Chapter III, and refer to those sources for more details. Let G be a finite group. Let EG → BG be a universal principal G-bundle. We think of EG as a contractible space on which G acts freely (on the right) with orbit space BG . Although EG and BG are infinite dimensional they can be chosen to have finite
1
Introduction
In this paper we investigate pseudofree homologically trivial actions of arbitrary finite groups on simply connected 4-manifolds. It turns out that there are surprisingly strong restrictions on the groups and on the actions, especially when one considers the fact that any finite group can act, even freely, on some simply connected 4-manifold. Recall that a finite group action is free if no nontrivial group element has a fixed point, pseudofree if the action is free on the complement of a discrete set, and semifree if it is free on the complement of the fixed point set of the whole group. An action is locally linear if each point has a neighborhood invariant under the isotropy group at the point on which the action is equivalent to a linear action of the isotropy group on some euclidean space. An an action is said to be homologically trivial if the induced action on all integral homology groups is the identity. Main Theorem. If a finite group G acts locally linearly, pseudofreely, and homologically trivially, on a closed, simply connected 4-manifold X , with b2 (X ) ≥ 3, then G is cyclic and acts semifreely, and the fixed point set consists of b2 (X ) + 2 isolated points. When the second Betti number b2 (X ) ≤ 2 other groups do arise. In the case of S 4 (corresponding to the case when b2 = 0),a number of other groups do come up, since any free linear action on on S 3 can be suspended to a pseudofree action, so a number of other groups arise. It turns out every group that acts pseudofreely on S 4 arises in this way. Most pseudofree actions on the complex projective plane CP 2 (b2 = 1) are in fact semifree, as follows from the classification of D. Wilczy´ nski [11], [12] and of I. Hambleton and R. Lee 1
动量四因素模型回归系数解释

动量四因素模型回归系数解释英文版The Momentum Four-Factor Model Regression Coefficient InterpretationIn finance, the momentum four-factor model is a widely used tool to analyze the returns of a stock or portfolio. This model incorporates four factors that are believed to drive stock returns: market risk, size, value, and momentum. By using regression analysis, investors can determine how these factors affect the returns of a particular stock or portfolio.The regression coefficients in the momentum four-factor model provide valuable insights into the relationship between the four factors and stock returns. The coefficient for market risk measures the sensitivity of a stock's returns to overall market movements.A coefficient greater than 1 indicates that the stock is more volatile than the market, while a coefficient less than 1 suggests that the stock is less volatile.The size coefficient reflects the impact of a stock's market capitalization on its returns. A positive coefficient indicates that smaller stocks tend to outperform larger stocks, while a negative coefficient suggests the opposite. The value coefficient measures the effect of a stock's price-to-book ratio on its returns. A positive coefficient implies that value stocks outperform growth stocks, while a negative coefficient indicates the opposite.Finally, the momentum coefficient captures the influence of a stock's past performance on its future returns. A positive coefficient suggests that stocks with strong recent performance continue to outperform, while a negative coefficient indicates that stocks with weak recent performance tend to underperform.In conclusion, the momentum four-factor model regression coefficients provide valuable information on how market risk, size, value, and momentum impact stock returns. By understanding and interpreting these coefficients, investors can make more informed decisions when constructing their portfolios.中文版动量四因素模型回归系数解释在金融领域,动量四因素模型是一种广泛使用的工具,用于分析股票或投资组合的回报。
人工智能化词汇12.17

常用英语词汇-andrew Ng课程intensity 强度Regression 回归Loss function 损失函数non-convex 非凸函数neural network 神经网络supervised learning 监督学习regression problem 回归问题处理的是连续的问题classification problem 分类问题discreet value 离散值support vector machines 支持向量机learning theory 学习理论learning algorithms 学习算法unsupervised learning 无监督学习gradient descent 梯度下降linear regression 线性回归Neural Network 神经网络gradient descent 梯度下降normal equationslinear algebra 线性代数superscript上标exponentiation 指数training set 训练集合training example 训练样本hypothesis 假设,用来表示学习算法的输出LMS algorithm “least mean squares 最小二乘法算法batch gradient descent 批量梯度下降constantly gradient descent 随机梯度下降iterative algorithm 迭代算法partial derivative 偏导数contour 等高线quadratic function 二元函数locally weighted regression局部加权回归underfitting欠拟合overfitting 过拟合non-parametric learning algorithms 无参数学习算法parametric learning algorithm 参数学习算法activation 激活值activation function 激活函数additive noise 加性噪声autoencoder 自编码器Autoencoders 自编码算法average firing rate 平均激活率average sum-of-squares error 均方差backpropagation 后向传播basis 基basis feature vectors 特征基向量batch gradient ascent 批量梯度上升法Bayesian regularization method 贝叶斯规则化方法Bernoulli random variable 伯努利随机变量bias term 偏置项binary classfication 二元分类class labels 类型标记concatenation 级联conjugate gradient 共轭梯度contiguous groups 联通区域convex optimization software 凸优化软件convolution 卷积cost function 代价函数covariance matrix 协方差矩阵DC component 直流分量decorrelation 去相关degeneracy 退化demensionality reduction 降维derivative 导函数diagonal 对角线diffusion of gradients 梯度的弥散eigenvalue 特征值eigenvector 特征向量error term 残差feature matrix 特征矩阵feature standardization 特征标准化feedforward architectures 前馈结构算法feedforward neural network 前馈神经网络feedforward pass 前馈传导fine-tuned 微调first-order feature 一阶特征forward pass 前向传导forward propagation 前向传播Gaussian prior 高斯先验概率generative model 生成模型gradient descent 梯度下降Greedy layer-wise training 逐层贪婪训练方法grouping matrix 分组矩阵Hadamard product 阿达马乘积Hessian matrix Hessian 矩阵hidden layer 隐含层hidden units 隐藏神经元Hierarchical grouping 层次型分组higher-order features 更高阶特征highly non-convex optimization problem高度非凸的优化问题histogram 直方图hyperbolic tangent 双曲正切函数hypothesis 估值,假设identity activation function 恒等激励函数IID 独立同分布illumination 照明inactive 抑制independent component analysis 独立成份分析input domains 输入域input layer 输入层intensity 亮度/灰度intercept term 截距KL divergence 相对熵KL divergence KL分散度k-Means K-均值learning rate 学习速率least squares 最小二乘法linear correspondence 线性响应linear superposition 线性叠加line-search algorithm 线搜索算法local mean subtraction 局部均值消减local optima 局部最优解logistic regression 逻辑回归loss function 损失函数low-pass filtering 低通滤波magnitude 幅值MAP 极大后验估计maximum likelihood estimation 极大似然估计mean 平均值MFCC Mel 倒频系数multi-class classification 多元分类neural networks 神经网络neuron 神经元Newton’s method 牛顿法non-convex function 非凸函数non-linear feature 非线性特征norm 范式norm bounded 有界范数norm constrained 范数约束normalization 归一化numerical roundoff errors 数值舍入误差numerically checking 数值检验numerically reliable 数值计算上稳定object detection 物体检测objective function 目标函数off-by-one error 缺位错误orthogonalization 正交化output layer 输出层overall cost function 总体代价函数over-complete basis 超完备基over-fitting 过拟合parts of objects 目标的部件part-whole decompostion 部分-整体分解PCA 主元分析penalty term 惩罚因子per-example mean subtraction 逐样本均值消减pooling 池化pretrain 预训练principal components analysis 主成份分析quadratic constraints 二次约束RBMs 受限Boltzman机reconstruction based models 基于重构的模型reconstruction cost 重建代价reconstruction term 重构项redundant 冗余reflection matrix 反射矩阵regularization 正则化regularization term 正则化项rescaling 缩放robust 鲁棒性run 行程second-order feature 二阶特征sigmoid activation function S型激励函数significant digits 有效数字singular value 奇异值singular vector 奇异向量smoothed L1 penalty 平滑的L1范数惩罚Smoothed topographic L1 sparsity penalty 平滑地形L1稀疏惩罚函数smoothing 平滑Softmax Regresson Softmax回归sorted in decreasing order 降序排列source features 源特征sparse autoencoder 消减归一化Sparsity 稀疏性sparsity parameter 稀疏性参数sparsity penalty 稀疏惩罚square function 平方函数squared-error 方差stationary 平稳性(不变性)stationary stochastic process 平稳随机过程step-size 步长值supervised learning 监督学习symmetric positive semi-definite matrix对称半正定矩阵symmetry breaking 对称失效tanh function 双曲正切函数the average activation 平均活跃度the derivative checking method 梯度验证方法the empirical distribution 经验分布函数the energy function 能量函数the Lagrange dual 拉格朗日对偶函数the log likelihood 对数似然函数the pixel intensity value 像素灰度值the rate of convergence 收敛速度topographic cost term 拓扑代价项topographic ordered 拓扑秩序transformation 变换translation invariant 平移不变性trivial answer 平凡解under-complete basis 不完备基unrolling 组合扩展unsupervised learning 无监督学习variance 方差vecotrized implementation 向量化实现vectorization 矢量化visual cortex 视觉皮层weight decay 权重衰减weighted average 加权平均值whitening 白化zero-mean 均值为零Accumulated error backpropagation 累积误差逆传播Activation Function 激活函数Adaptive Resonance Theory/ART 自适应谐振理论Addictive model 加性学习Adversarial Networks 对抗网络Affine Layer 仿射层Affinity matrix 亲和矩阵Agent 代理/ 智能体Algorithm 算法Alpha-beta pruning α-β剪枝Anomaly detection 异常检测Approximation 近似Area Under ROC Curve/AUC Roc 曲线下面积Artificial General Intelligence/AGI 通用人工智能Artificial Intelligence/AI 人工智能Association analysis 关联分析Attention mechanism 注意力机制Attribute conditional independence assumption属性条件独立性假设Attribute space 属性空间Attribute value 属性值Autoencoder 自编码器Automatic speech recognition 自动语音识别Automatic summarization 自动摘要Average gradient 平均梯度Average-Pooling 平均池化Backpropagation Through Time 通过时间的反向传播Backpropagation/BP 反向传播Base learner 基学习器Base learning algorithm 基学习算法Batch Normalization/BN 批量归一化Bayes decision rule 贝叶斯判定准则Bayes Model Averaging/BMA 贝叶斯模型平均Bayes optimal classifier 贝叶斯最优分类器Bayesian decision theory 贝叶斯决策论Bayesian network 贝叶斯网络Between-class scatter matrix 类间散度矩阵Bias 偏置/ 偏差Bias-variance decomposition 偏差-方差分解Bias-Variance Dilemma 偏差–方差困境Bi-directional Long-Short Term Memory/Bi-LSTM 双向长短期记忆Binary classification 二分类Binomial test 二项检验Bi-partition 二分法Boltzmann machine 玻尔兹曼机Bootstrap sampling 自助采样法/可重复采样Bootstrapping 自助法Break-Event Point/BEP 平衡点Calibration 校准Cascade-Correlation 级联相关Categorical attribute 离散属性Class-conditional probability 类条件概率Classification and regression tree/CART 分类与回归树Classifier 分类器Class-imbalance 类别不平衡Closed -form 闭式Cluster 簇/类/集群Cluster analysis 聚类分析Clustering 聚类Clustering ensemble 聚类集成Co-adapting 共适应Coding matrix 编码矩阵COLT 国际学习理论会议Committee-based learning 基于委员会的学习Competitive learning 竞争型学习Component learner 组件学习器Comprehensibility 可解释性Computation Cost 计算成本Computational Linguistics 计算语言学Computer vision 计算机视觉Concept drift 概念漂移Concept Learning System /CLS 概念学习系统Conditional entropy 条件熵Conditional mutual information 条件互信息Conditional Probability Table/CPT 条件概率表Conditional random field/CRF 条件随机场Conditional risk 条件风险Confidence 置信度Confusion matrix 混淆矩阵Connection weight 连接权Connectionism 连结主义Consistency 一致性/相合性Contingency table 列联表Continuous attribute 连续属性Convergence 收敛Conversational agent 会话智能体Convex quadratic programming 凸二次规划Convexity 凸性Convolutional neural network/CNN 卷积神经网络Co-occurrence 同现Correlation coefficient 相关系数Cosine similarity 余弦相似度Cost curve 成本曲线Cost Function 成本函数Cost matrix 成本矩阵Cost-sensitive 成本敏感Cross entropy 交叉熵Cross validation 交叉验证Crowdsourcing 众包Curse of dimensionality 维数灾难Cut point 截断点Cutting plane algorithm 割平面法Data mining 数据挖掘Data set 数据集Decision Boundary 决策边界Decision stump 决策树桩Decision tree 决策树/判定树Deduction 演绎Deep Belief Network 深度信念网络DeepConvolutional Generative Adversarial Network DCGAN 深度卷积生成对抗网络Deep learning 深度学习Deep neural network/DNN 深度神经网络Deep Q-Learning 深度Q 学习Deep Q-Network 深度Q 网络Density estimation 密度估计Density-based clustering 密度聚类Differentiable neural computer 可微分神经计算机Dimensionality reduction algorithm 降维算法Directed edge 有向边Disagreement measure 不合度量Discriminative model 判别模型Discriminator 判别器Distance measure 距离度量Distance metric learning 距离度量学习Distribution 分布Divergence 散度Diversity measure 多样性度量/差异性度量Domain adaption 领域自适应Downsampling 下采样D-separation (Directed separation)有向分离Dual problem 对偶问题Dummy node 哑结点Dynamic Fusion 动态融合Dynamic programming 动态规划Eigenvalue decomposition 特征值分解Embedding 嵌入Emotional analysis 情绪分析Empirical conditional entropy 经验条件熵Empirical entropy 经验熵Empirical error 经验误差Empirical risk 经验风险End-to-End 端到端Energy-based model 基于能量的模型Ensemble learning 集成学习Ensemble pruning 集成修剪Error Correcting Output Codes/ECOC 纠错输出码Error rate 错误率Error-ambiguity decomposition 误差-分歧分解Euclidean distance 欧氏距离Evolutionary computation 演化计算Expectation-Maximization 期望最大化Expected loss 期望损失Exploding Gradient Problem 梯度爆炸问题Exponential loss function 指数损失函数Extreme Learning Machine/ELM 超限学习机Factorization 因子分解False negative 假负类False positive 假正类False Positive Rate/FPR 假正例率Feature engineering 特征工程Feature selection 特征选择Feature vector 特征向量Featured Learning 特征学习Feedforward Neural Networks/FNN 前馈神经网络Fine-tuning 微调Flipping output 翻转法Fluctuation 震荡Forward stagewise algorithm 前向分步算法Frequentist 频率主义学派Full-rank matrix 满秩矩阵Functional neuron 功能神经元Gain ratio 增益率Game theory 博弈论Gaussian kernel function 高斯核函数Gaussian Mixture Model 高斯混合模型General Problem Solving 通用问题求解Generalization 泛化Generalization error 泛化误差Generalization error bound 泛化误差上界Generalized Lagrange function 广义拉格朗日函数Generalized linear model 广义线性模型Generalized Rayleigh quotient 广义瑞利商Generative Adversarial Networks/GAN 生成对抗网络Generative Model 生成模型Generator 生成器Genetic Algorithm/GA 遗传算法Gibbs sampling 吉布斯采样Gini index 基尼指数Global minimum 全局最小Global Optimization 全局优化Gradient boosting 梯度提升Gradient Descent 梯度下降Graph theory 图论Ground-truth 真相/真实Hard margin 硬间隔Hard voting 硬投票Harmonic mean 调和平均Hesse matrix 海塞矩阵Hidden dynamic model 隐动态模型Hidden layer 隐藏层Hidden Markov Model/HMM 隐马尔可夫模型Hierarchical clustering 层次聚类Hilbert space 希尔伯特空间Hinge loss function 合页损失函数Hold-out 留出法Homogeneous 同质Hybrid computing 混合计算Hyperparameter 超参数Hypothesis 假设Hypothesis test 假设验证ICML 国际机器学习会议Improved iterative scaling/IIS 改进的迭代尺度法Incremental learning 增量学习Independent and identically distributed/i.i.d.独立同分布Independent Component Analysis/ICA 独立成分分析Indicator function 指示函数Individual learner 个体学习器Induction 归纳Inductive bias 归纳偏好Inductive learning 归纳学习Inductive Logic Programming/ILP 归纳逻辑程序设计Information entropy 信息熵Information gain 信息增益Input layer 输入层Insensitive loss 不敏感损失Inter-cluster similarity 簇间相似度International Conference for Machine Learning/ICML 国际机器学习大会Intra-cluster similarity 簇内相似度Intrinsic value 固有值Isometric Mapping/Isomap 等度量映射Isotonic regression 等分回归Iterative Dichotomiser 迭代二分器Kernel method 核方法Kernel trick 核技巧Kernelized Linear Discriminant Analysis/KLDA核线性判别分析K-fold cross validation k 折交叉验证/k 倍交叉验证K-Means Clustering K –均值聚类K-Nearest Neighbours Algorithm/KNN K近邻算法Knowledge base 知识库Knowledge Representation 知识表征Label space 标记空间Lagrange duality 拉格朗日对偶性Lagrange multiplier 拉格朗日乘子Laplace smoothing 拉普拉斯平滑Laplacian correction 拉普拉斯修正Latent Dirichlet Allocation 隐狄利克雷分布Latent semantic analysis 潜在语义分析Latent variable 隐变量Lazy learning 懒惰学习Learner 学习器Learning by analogy 类比学习Learning rate 学习率Learning Vector Quantization/LVQ 学习向量量化Least squares regression tree 最小二乘回归树Leave-One-Out/LOO 留一法linear chain conditional random field线性链条件随机场Linear Discriminant Analysis/LDA 线性判别分析Linear model 线性模型Linear Regression 线性回归Link function 联系函数Local Markov property 局部马尔可夫性Local minimum 局部最小Log likelihood 对数似然Log odds/logit 对数几率Logistic Regression Logistic 回归Log-likelihood 对数似然Log-linear regression 对数线性回归Long-Short Term Memory/LSTM 长短期记忆Loss function 损失函数Machine translation/MT 机器翻译Macron-P 宏查准率Macron-R 宏查全率Majority voting 绝对多数投票法Manifold assumption 流形假设Manifold learning 流形学习Margin theory 间隔理论Marginal distribution 边际分布Marginal independence 边际独立性Marginalization 边际化Markov Chain Monte Carlo/MCMC马尔可夫链蒙特卡罗方法Markov Random Field 马尔可夫随机场Maximal clique 最大团Maximum Likelihood Estimation/MLE极大似然估计/极大似然法Maximum margin 最大间隔Maximum weighted spanning tree 最大带权生成树Max-Pooling 最大池化Mean squared error 均方误差Meta-learner 元学习器Metric learning 度量学习Micro-P 微查准率Micro-R 微查全率Minimal Description Length/MDL 最小描述长度Minimax game 极小极大博弈Misclassification cost 误分类成本Mixture of experts 混合专家Momentum 动量Moral graph 道德图/端正图Multi-class classification 多分类Multi-document summarization 多文档摘要Multi-layer feedforward neural networks多层前馈神经网络Multilayer Perceptron/MLP 多层感知器Multimodal learning 多模态学习Multiple Dimensional Scaling 多维缩放Multiple linear regression 多元线性回归Multi-response Linear Regression /MLR多响应线性回归Mutual information 互信息Naive bayes 朴素贝叶斯Naive Bayes Classifier 朴素贝叶斯分类器Named entity recognition 命名实体识别Nash equilibrium 纳什均衡Natural language generation/NLG 自然语言生成Natural language processing 自然语言处理Negative class 负类Negative correlation 负相关法Negative Log Likelihood 负对数似然Neighbourhood Component Analysis/NCA近邻成分分析Neural Machine Translation 神经机器翻译Neural Turing Machine 神经图灵机Newton method 牛顿法NIPS 国际神经信息处理系统会议No Free Lunch Theorem/NFL 没有免费的午餐定理Noise-contrastive estimation 噪音对比估计Nominal attribute 列名属性Non-convex optimization 非凸优化Nonlinear model 非线性模型Non-metric distance 非度量距离Non-negative matrix factorization 非负矩阵分解Non-ordinal attribute 无序属性Non-Saturating Game 非饱和博弈Norm 范数Normalization 归一化Nuclear norm 核范数Numerical attribute 数值属性Letter OObjective function 目标函数Oblique decision tree 斜决策树Occam’s razor 奥卡姆剃刀Odds 几率Off-Policy 离策略One shot learning 一次性学习One-Dependent Estimator/ODE 独依赖估计On-Policy 在策略Ordinal attribute 有序属性Out-of-bag estimate 包外估计Output layer 输出层Output smearing 输出调制法Overfitting 过拟合/过配Oversampling 过采样Paired t-test 成对t 检验Pairwise 成对型Pairwise Markov property 成对马尔可夫性Parameter 参数Parameter estimation 参数估计Parameter tuning 调参Parse tree 解析树Particle Swarm Optimization/PSO 粒子群优化算法Part-of-speech tagging 词性标注Perceptron 感知机Performance measure 性能度量Plug and Play Generative Network 即插即用生成网络Plurality voting 相对多数投票法Polarity detection 极性检测Polynomial kernel function 多项式核函数Pooling 池化Positive class 正类Positive definite matrix 正定矩阵Post-hoc test 后续检验Post-pruning 后剪枝potential function 势函数Precision 查准率/准确率Prepruning 预剪枝Principal component analysis/PCA 主成分分析Principle of multiple explanations 多释原则Prior 先验Probability Graphical Model 概率图模型Proximal Gradient Descent/PGD 近端梯度下降Pruning 剪枝Pseudo-label 伪标记Quantized Neural Network 量子化神经网络Quantum computer 量子计算机Quantum Computing 量子计算Quasi Newton method 拟牛顿法Radial Basis Function/RBF 径向基函数Random Forest Algorithm 随机森林算法Random walk 随机漫步Recall 查全率/召回率Receiver Operating Characteristic/ROC受试者工作特征Rectified Linear Unit/ReLU 线性修正单元Recurrent Neural Network 循环神经网络Recursive neural network 递归神经网络Reference model 参考模型Regression 回归Regularization 正则化Reinforcement learning/RL 强化学习Representation learning 表征学习Representer theorem 表示定理reproducing kernel Hilbert space/RKHS再生核希尔伯特空间Re-sampling 重采样法Rescaling 再缩放Residual Mapping 残差映射Residual Network 残差网络Restricted Boltzmann Machine/RBM 受限玻尔兹曼机Restricted Isometry Property/RIP 限定等距性Re-weighting 重赋权法Robustness 稳健性/鲁棒性Root node 根结点Rule Engine 规则引擎Rule learning 规则学习Saddle point 鞍点Sample space 样本空间Sampling 采样Score function 评分函数Self-Driving 自动驾驶Self-Organizing Map/SOM 自组织映射Semi-naive Bayes classifiers 半朴素贝叶斯分类器Semi-Supervised Learning 半监督学习semi-Supervised Support Vector Machine半监督支持向量机Sentiment analysis 情感分析Separating hyperplane 分离超平面Sigmoid function Sigmoid 函数Similarity measure 相似度度量Simulated annealing 模拟退火Simultaneous localization and mapping同步定位与地图构建Singular Value Decomposition 奇异值分解Slack variables 松弛变量Smoothing 平滑Soft margin 软间隔Soft margin maximization 软间隔最大化Soft voting 软投票Sparse representation 稀疏表征Sparsity 稀疏性Specialization 特化Spectral Clustering 谱聚类Speech Recognition 语音识别Splitting variable 切分变量Squashing function 挤压函数Stability-plasticity dilemma 可塑性-稳定性困境Statistical learning 统计学习Status feature function 状态特征函Stochastic gradient descent 随机梯度下降Stratified sampling 分层采样Structural risk 结构风险Structural risk minimization/SRM 结构风险最小化Subspace 子空间Supervised learning 监督学习/有导师学习support vector expansion 支持向量展式Support Vector Machine/SVM 支持向量机Surrogat loss 替代损失Surrogate function 替代函数Symbolic learning 符号学习Symbolism 符号主义Synset 同义词集T-Distribution Stochastic Neighbour Embedding t-SNE T –分布随机近邻嵌入Tensor 张量Tensor Processing Units/TPU 张量处理单元The least square method 最小二乘法Threshold 阈值Threshold logic unit 阈值逻辑单元Threshold-moving 阈值移动Time Step 时间步骤Tokenization 标记化Training error 训练误差Training instance 训练示例/训练例Transductive learning 直推学习Transfer learning 迁移学习Treebank 树库Tria-by-error 试错法True negative 真负类True positive 真正类True Positive Rate/TPR 真正例率Turing Machine 图灵机Twice-learning 二次学习Underfitting 欠拟合/欠配Undersampling 欠采样Understandability 可理解性Unequal cost 非均等代价Unit-step function 单位阶跃函数Univariate decision tree 单变量决策树Unsupervised learning 无监督学习/无导师学习Unsupervised layer-wise training 无监督逐层训练Upsampling 上采样Vanishing Gradient Problem 梯度消失问题Variational inference 变分推断VC Theory VC维理论Version space 版本空间Viterbi algorithm 维特比算法Von Neumann architecture 冯·诺伊曼架构Wasserstein GAN/WGAN Wasserstein生成对抗网络Weak learner 弱学习器Weight 权重Weight sharing 权共享Weighted voting 加权投票法Within-class scatter matrix 类内散度矩阵Word embedding 词嵌入Word sense disambiguation 词义消歧Zero-data learning 零数据学习Zero-shot learning 零次学习approximations近似值arbitrary随意的affine仿射的arbitrary任意的amino acid氨基酸amenable经得起检验的axiom公理,原则abstract提取architecture架构,体系结构;建造业absolute绝对的arsenal军火库assignment分配algebra线性代数asymptotically无症状的appropriate恰当的bias偏差brevity简短,简洁;短暂[800 ] broader广泛briefly简短的batch批量convergence 收敛,集中到一点convex凸的contours轮廓constraint约束constant常理commercial商务的complementarity补充coordinate ascent同等级上升clipping剪下物;剪报;修剪component分量;部件continuous连续的covariance协方差canonical正规的,正则的concave非凸的corresponds相符合;相当;通信corollary推论concrete具体的事物,实在的东西cross validation交叉验证correlation相互关系convention约定cluster一簇centroids 质心,形心converge收敛computationally计算(机)的calculus计算derive获得,取得dual二元的duality二元性;二象性;对偶性derivation求导;得到;起源denote预示,表示,是…的标志;意味着,[逻]指称divergence 散度;发散性dimension尺度,规格;维数dot小圆点distortion变形density概率密度函数discrete离散的discriminative有识别能力的diagonal对角dispersion分散,散开determinant决定因素disjoint不相交的encounter遇到ellipses椭圆equality等式extra额外的empirical经验;观察ennmerate例举,计数exceed超过,越出expectation期望efficient生效的endow赋予explicitly清楚的exponential family指数家族equivalently等价的feasible可行的forary初次尝试finite有限的,限定的forgo摒弃,放弃fliter过滤frequentist最常发生的forward search前向式搜索formalize使定形generalized归纳的generalization概括,归纳;普遍化;判断(根据不足)guarantee保证;抵押品generate形成,产生geometric margins几何边界gap裂口generative生产的;有生产力的heuristic启发式的;启发法;启发程序hone怀恋;磨hyperplane超平面initial最初的implement执行intuitive凭直觉获知的incremental增加的intercept截距intuitious直觉instantiation例子indicator指示物,指示器interative重复的,迭代的integral积分identical相等的;完全相同的indicate表示,指出invariance不变性,恒定性impose把…强加于intermediate中间的interpretation解释,翻译joint distribution联合概率lieu替代logarithmic对数的,用对数表示的latent潜在的Leave-one-out cross validation留一法交叉验证magnitude巨大mapping绘图,制图;映射matrix矩阵mutual相互的,共同的monotonically单调的minor较小的,次要的multinomial多项的multi-class classification二分类问题nasty讨厌的notation标志,注释naïve朴素的obtain得到oscillate摆动optimization problem最优化问题objective function目标函数optimal最理想的orthogonal(矢量,矩阵等)正交的orientation方向ordinary普通的occasionally偶然的partial derivative偏导数property性质proportional成比例的primal原始的,最初的permit允许pseudocode伪代码permissible可允许的polynomial多项式preliminary预备precision精度perturbation 不安,扰乱poist假定,设想positive semi-definite半正定的parentheses圆括号posterior probability后验概率plementarity补充pictorially图像的parameterize确定…的参数poisson distribution柏松分布pertinent相关的quadratic二次的quantity量,数量;分量query疑问的regularization使系统化;调整reoptimize重新优化restrict限制;限定;约束reminiscent回忆往事的;提醒的;使人联想…的(of)remark注意random variable随机变量respect考虑respectively各自的;分别的redundant过多的;冗余的susceptible敏感的stochastic可能的;随机的symmetric对称的sophisticated复杂的spurious假的;伪造的subtract减去;减法器simultaneously同时发生地;同步地suffice满足scarce稀有的,难得的split分解,分离subset子集statistic统计量successive iteratious连续的迭代scale标度sort of有几分的squares平方trajectory轨迹temporarily暂时的terminology专用名词tolerance容忍;公差thumb翻阅threshold阈,临界theorem定理tangent正弦unit-length vector单位向量valid有效的,正确的variance方差variable变量;变元vocabulary词汇valued经估价的;宝贵的wrapper包装总计1038词汇。
高斯朴素贝叶斯训练集精确度的英语

高斯朴素贝叶斯训练集精确度的英语Gaussian Naive Bayes (GNB) is a popular machine learning algorithm used for classification tasks. It is particularly well-suited for text classification, spam filtering, and recommendation systems. However, like any other machine learning algorithm, GNB's performance heavily relies on the quality of the training data. In this essay, we will delve into the factors that affect the training set accuracy of Gaussian Naive Bayes and explore potential solutions to improve its performance.One of the key factors that influence the training set accuracy of GNB is the quality and quantity of the training data. In order for the algorithm to make accurate predictions, it needs to be trained on a diverse and representative dataset. If the training set is too small or biased, the model may not generalize well to new, unseen data. This can result in low training set accuracy and poor performance in real-world applications. Therefore, it is crucial to ensure that the training data is comprehensive and well-balanced across different classes.Another factor that can impact the training set accuracy of GNB is the presence of irrelevant or noisy features in the dataset. When the input features contain irrelevant information or noise, it can hinder the algorithm's ability to identify meaningful patterns and make accurate predictions. To address this issue, feature selection and feature engineering techniques can be employed to filter out irrelevant features and enhance the discriminative power of the model. Byselecting the most informative features and transforming them appropriately, we can improve the training set accuracy of GNB.Furthermore, the assumption of feature independence in Gaussian Naive Bayes can also affect its training set accuracy. Although the 'naive' assumption of feature independence simplifies the model and makes it computationally efficient, it may not hold true in real-world datasets where features are often correlated. When features are not independent, it can lead to biased probability estimates and suboptimal performance. To mitigate this issue, techniques such as feature extraction and dimensionality reduction can be employed to decorrelate the input features and improve the training set accuracy of GNB.In addition to the aforementioned factors, the choice of hyperparameters and model tuning can also impact the training set accuracy of GNB. Hyperparameters such as the smoothing parameter (alpha) and the covariance type in the Gaussian distribution can significantly influence the model's performance. Therefore, it is important to carefully tune these hyperparameters through cross-validation andgrid search to optimize the training set accuracy of GNB. By selecting the appropriate hyperparameters, we can ensure that the model is well-calibrated and achieves high accuracy on the training set.Despite the challenges and limitations associated with GNB, there are several strategies that can be employed to improve its training set accuracy. By curating a high-quality training dataset, performing feature selection and engineering, addressing feature independence assumptions, and tuning model hyperparameters, we can enhance the performance of GNB and achieve higher training set accuracy. Furthermore, it is important to continuously evaluate and validate the model on unseen data to ensure that it generalizes well and performs robustly in real-world scenarios. By addressing these factors and adopting best practices in model training and evaluation, we can maximize the training set accuracy of Gaussian Naive Bayes and unleash its full potential in various applications.。
漫谈微分几何、多复变函数与代数几何(Differential geometry, functions

漫谈微分几何、多复变函数与代数几何(Differential geometry, functions of complex variable and algebraic geometry)Differential geometry and tensor analysis, developed with the development of differential geometry, are the basic tools for mastering general relativity. Because general relativity's success, to always obscure differential geometry has become one of the central discipline of mathematics.Since the invention of differential calculus, the birth of differential geometry was born. But the work of Euler, Clairaut and Monge really made differential geometry an independent discipline. In the work of geodesy, Euler has gradually obtained important research, and obtained the famous Euler formula for the calculation of normal curvature. The Clairaut curve of the curvature and torsion, Monge published "analysis is applied to the geometry of the loose leaf paper", the important properties of curves and surfaces are represented by differential equations, which makes the development of classical differential geometry to reach a peak. Gauss in the study of geodesic, through complicated calculation, in 1827 found two main curvature surfaces and its product in the periphery of the Euclidean shape of the space not only depends on its first fundamental form, the result is Gauss proudly called the wonderful theorem, created from the intrinsic geometry. The free surface of space from the periphery, the surface itself as a space to study. In 1854, Riemann made the hypothesis about geometric foundation, and extended the intrinsic geometry of Gauss in 2 dimensional curved surface, thus developing n-dimensional Riemann geometry, with the development of complex functions. A group of excellentmathematicians extended the research objects of differential geometry to complex manifolds and extended them to the complex analytic space theory including singularities. Each step of differential geometry faces not only the deepening of knowledge, but also the continuous expansion of the field of knowledge. Here, differential geometry and complex functions, Lie group theory, algebraic geometry, and PDE all interact profoundly with one another. Mathematics is constantly dividing and blending with each other.By shining the charming glory and the differential geometric function theory of several complex variables, unit circle and the upper half plane (the two conformal mapping establishment) defined on Poincare metric, complex function theory and the differential geometric relationships can be seen distinctly. Poincare metric is conformal invariant. The famous Schwarz theorem can be explained as follows: the Poincare metric on the unit circle does not increase under analytic mapping; if and only if the mapping is a fractional linear transformation, the Poincare metric does not change Poincare. Applying the hyperbolic geometry of Poincare metric, we can easily prove the famous Picard theorem. The proof of Picard theorem to modular function theory is hard to use, if using the differential geometric point of view, can also be in a very simple way to prove. Differential geometry permeates deep into the theory of complex functions. In the theory of multiple complex functions, the curvature of the real differential geometry and other series of calculations are followed by the analysis of the region definition metric of the complex affine space. In complex situations, all of the singular discrete distribution, and in more complex situations, because of the famous Hartogsdevelopment phenomenon, all isolated singularities are engulfed by a continuous region even in singularity formation is often destroyed, only the formation of real codimension 1 manifold can avoid the bad luck. But even this situation requires other restrictions to ensure safety". The singular properties of singularities in the theory of functions of complex functions make them destined to be manifolds. In 1922, Bergman introduced the famous Bergman kernel function, the more complex function or Weyl said its era, in addition to the famous Hartogs, Poincare, Levi of Cousin and several predecessors almost no substantive progress, injected a dynamic Bergman work will undoubtedly give this dead area. In many complex function domains in the Bergman metric metric in the one-dimensional case is the unit circle and Poincare on the upper half plane of the Poincare, which doomed the importance of the work of Bergman.The basic object of algebraic geometry is the properties of the common zeros (algebraic families) of any dimension, affine space, or algebraic equations of a projective space (defined equations),The definitions of algebraic clusters, the coefficients of equations, and the domains in which the points of an algebraic cluster are located are called base domains. An irreducible algebraic variety is a finite sub extension of its base domain. In our numerical domain, the linear space is the extension of the base field in the number field, and the dimension of the linear space is the number of the expansion. From this point of view, algebraic geometry can be viewed as a study of finite extension fields. The properties of algebraic clusters areclosely related to their base domains. The algebraic domain of complex affine space or complex projective space, the research process is not only a large number of concepts and differential geometry and complex function theory and applied to a large number of coincidence, the similar tools in the process of research. Every step of the complex manifold and the complex analytic space has the same influence on these subjects. Many masters in related fields, although they seem to study only one field, have consequences for other areas. For example: the Lerey study of algebraic topology that it has little effect on layer, in algebraic topology, but because of Serre, Weil and H? Cartan (E? Cartan, eldest son) introduction, has a profound impact on algebraic geometry and complex function theory. Chern studies the categories of Hermite spaces, but it also affects algebraic geometry, differential geometry and complex functions. Hironaka studies the singular point resolution in algebraic geometry, but the modification of complex manifold to complex analytic space and blow up affect the theory of complex analytic space. Yau proves that the Calabi conjecture not only affects algebraic geometry and differential geometry, but also affects classical general relativity. At the same time, we can see the important position of nonlinear ordinary differential equations and partial differential equations in differential geometry. Cartan study of symmetric Riemann space, the classification theorem is important, given 1, 2 and 3 dimensional space of a Homogeneous Bounded Domain complete classification, prove that they are all homogeneous symmetric domains at the same time, he guessed: This is also true in the n-dimensional equivalent relation. In 1959, Piatetski-Shapiro has two counterexample and find the domain theory of automorphic function study in symmetry, in the 4 and 5dimensional cases each find a homogeneous bounded domain, which is not a homogeneous symmetric domain, the domain he named Siegel domain, to commemorate the profound work on Siegel in 1943 of automorphic function. The results of Piatetski-Shapiro has profound impact on the theory of complex variable functions and automorphic function theory, and have a profound impact on the symmetry space theory and a series of topics. As we know, Cartan transforms the study of symmetric spaces into the study of Lie groups and Lie algebras, which is directly influenced by Klein and greatly develops the initial idea of Klein. Then it is Cartan developed the concept of Levi-Civita connection, the development of differential geometry in general contact theory, isomorphic mapping through tangent space at each point on the manifold, realize the dream of Klein and greatly promote the development of differential geometry. Cartan is the same, and concluded that the importance of the research in the holonomy manifold twists and turns, finally after his death in thirty years has proved to be correct. Here, we see the vast beauty of differential geometry.As we know, geodesic ties are associated with ODE (ordinary differential equations), minimal surfaces and high dimensional submanifolds are associated with PDE (partial differential equations). These equations are nonlinear equations, so they have high requirements for analysis. Complex PDE and complex analysis the relationship between Cauchy-Riemann equations coupling the famous function theory, in the complex case, the Cauchy- Riemann equations not only deepen the unprecedented contact and the qualitative super Cauchy-Riemann equations (the number of variables is greater than the number of equations) led to a strange phenomenon. This makes PDE and the theory ofmultiple complex functions closely integrated with differential geometry.Most of the scholars have been studying the differential geometry of the intrinsic geometry of the Gauss and Riemann extremely deep stun, by Cartan's method of moving frames is beautiful and concise dumping, by Chern's theory of characteristic classes of the broad and profound admiration, Yau deep exquisite geometric analysis skills to deter.When the young Chern faced the whole differentiation, he said he was like a mountain facing the shining golden light, but he couldn't reach the summit at one time. But then he was cast as a master in this field before Hopf and Weil.If the differential geometry Cartan development to gradually change the general relativistic geometric model, then the differential geometry of Chern et al not only affect the continuation of Cartan and to promote the development of fiber bundle in the form of gauge field theory. Differential geometry is still closely bound up with physics as in the age of Einstein and continues to acquire research topics from physicsWhy does the three-dimensional sphere not give flatness gauge, but can give conformal flatness gauge? Because 3D balls and other dimension as the ball to establish flat space isometric mapping, so it is impossible to establish a flatness gauge; and n-dimensional balls are usually single curvature space, thus can establish a conformal flat metric. In differential geometry, isometry means that the distance between the points on the manifold before and after the mapping remains the same. Whena manifold is equidistant from a flat space, the curvature of its Riemann cross section is always zero. Since the curvature of all spheres is positive constant, the n-dimensional sphere and other manifolds whose sectional curvature is nonzero can not be assigned to local flatness gauge.But there are locally conformally flat manifolds for this concept, two gauge G and G, if G=exp{is called G, P}? G between a and G transform is a conformal transformation. Weyl conformal curvature tensor remains unchanged under conformal transformation. It is a tensor field of (1,3) type on a manifold. When the Weyl conformal curvature tensor is zero, the curvature tensor of the manifold can be represented by the Ricci curvature tensor and the scalar curvature, so Penrose always emphasizes the curvature =Ricci+Weyl.The metric tensor g of an n-dimensional Riemann manifold is conformally equivalent to the flatness gauge locally, and is called conformally flat manifold. All Manifolds (constant curvature manifolds) whose curvature is constant are conformally flat, so they can be given conformal conformal metric. And all dimensions of the sphere (including thethree-dimensional sphere) are manifold of constant curvature, so they must be given conformal conformal metric. Conversely, conformally flat manifolds are not necessarily manifolds of constant curvature. But a wonderful result related to Einstein manifolds can make up for this regret: conformally conformally Einstein manifolds over 3 dimensions must be manifolds of constant curvature. That is to say, if we want conformally conformally flat manifolds to be manifolds of constant curvature, we must call Ric= lambda g, and this is thedefinition of Einstein manifolds. In the formula, Ric is the Ricci curvature tensor, G is the metric tensor, and lambda is constant. The scalar curvature S=m of Einstein manifolds is constant. Moreover, if S is nonzero, there is no nonzero parallel tangent vector field over it. Einstein introduction of the cosmological constant. So he missed the great achievements that the expansion of the universe, so Hubble is successful in the official career; but the vacuum gravitational field equation of cosmological term with had a Einstein manifold, which provides a new stage for mathematicians wit.For the 3 dimensional connected Einstein manifold, even if does not require the conformal flat, it is also the automatic constant curvature manifolds, other dimensions do not set up this wonderful nature, I only know that this is the tensor analysis summer learning, the feeling is a kind of enjoyment. The sectional curvature in the real manifold is different from the curvature of the Holomorphic cross section in the Kahler manifold, and thus produces different results. If the curvature of holomorphic section is constant, the Ricci curvature of the manifold must be constant, so it must be Einstein manifold, called Kahler- Einstein manifold, Kahler. Kahler manifolds are Kahler- Einstein manifolds, if and only if they are Riemann manifolds, Einstein manifolds. N dimensional complex vector space, complex projective space, complex torus and complex hyperbolic space are Kahler- and Einstein manifolds. The study of Kahler-Einstein manifolds becomes the intellectual enjoyment of geometer.Let's go back to an important result of isometric mapping.In this paper, we consider the isometric mapping between M and N and the mapping of the cut space between the two Riemann manifolds, take P at any point on M, and select two non tangent tangent vectors in its tangent space, and obtain its sectional curvature. In the mapping, the two tangent vectors on the P point and its tangent space are transformed into two other tangent vectors under the mapping, and the sectional curvature of the vector is also obtained. If the mapping is isometric mapping, then the curvature of the two cross sections is equal. Or, to be vague, isometric mapping does not change the curvature of the section.Conversely, if the arbitrary points are set, the curvature of the section does not change in nature, then the mapping is not isometric mapping The answer was No. Even in thethree-dimensional Euclidean space on the surface can not set up this property. In some cases, the limit of the geodesic line must be added, and the properties of the Jacobi field can be used to do so. This is the famous Cartan isometry theorem. This theorem is a wonderful application of the Jacobi field. Its wide range of promotion is made by Ambrose and Hicks, known as the Cartan-Ambrose-Hicks theorem.Differential geometry is full of infinite charm. We classify pseudo-Riemannian spaces by using Weyl conformal curvature tensor, which can be classified by Ricci curvature tensor, or classified into 9 types by Bianchi. And these things are all can be attributed to the study of differential geometry, this distant view Riemann and slightly closer to the Klein point of the perfect combination, it can be seen that the great wisdom Cartan, here you can see the profound influence of Einstein.From the Hermite symmetry space to the Kahler-Hodge manifold, differential geometry is not only closely linked with the Lie group, but also connected with algebra, geometry and topologyThink of the great 1895 Poicare wrote the great "position analysis" was founded combination topology unabashedly said differential geometry in high dimensional space is of little importance to this subject, he said: "the home has beautiful scenery, where Xuyuan for." (Chern) topology is the beauty of the home. Why do you have to work hard to compute the curvature of surfaces or even manifolds of high dimensions? But this versatile mathematician is wrong, but we can not say that the mathematical genius no major contribution to differential geometry? Can not. Let's see today's close relation between differential geometry and topology, we'll see. When is a closed form the proper form? The inverse of the Poicare lemma in the region of the homotopy point (the single connected region) tells us that it is automatically established. In the non simply connected region is de famous Rham theorem tells us how to set up, that is the integral differential form in all closed on zero.Even in the field of differential geometry ignored by Poicare, he is still in a casual way deeply affected by the subject, or rather is affecting the whole mathematics.The nature of any discipline that seeks to be generalized after its creation, as is differential geometry. From the curvature, Euclidean curvature of space straight to zero, geometry extended to normal curvature number (narrow Riemann space) andnegative constant space (Lobachevskii space), we know that the greatness of non Euclidean geometry is that it not only independent of the fifth postulate and other alternative to the new geometry. It can be the founder of triangle analysis on it. But the famous mathematician Milnor said that before differential geometry went into non Euclidean geometry, non Euclidean geometry was only the torso with no hands and no feet. The non Euclidean geometry is born only when the curvature is computed uniformly after the metric is defined. In his speech in 1854, Riemann wrote only one formula: that is, this formula unifies the positive curvature, negative curvature and zero curvature geometry. Most people think that the formula for "Riemann" is based on intuition. In fact, later people found the draft paper that he used to calculate the formula. Only then did he realize that talent should be diligent. Riemann has explored the curvature of manifolds of arbitrary curvature of any dimension, but the quantitative calculations go beyond the mathematical tools of that time, and he can only write the unified formula for manifolds of constant curvature. But we know,Even today, this result is still important, differential geometry "comparison theorem" a multitude of names are in constant curvature manifolds for comparison model.When Riemann had considered two differential forms the root of two, this is what we are familiar with the Riemann metric Riemannnian, derived from geometry, he specifically mentioned another case, is the root of four four differential forms (equivalent to four yuan product and four times square). This is the contact and the difference between the two. But he saidthat for this situation and the previous case, the study does not require substantially different methods. It also says that such studies are time consuming and that new insights cannot be added to space, and the results of calculations lack geometric meaning. So Riemann studied only what is now called Riemann metric. Why are future generations of Finsler interested in promoting the Riemann's not wanting to study? It may be that mathematicians are so good that they become a hobby. Cartan in Finsler geometry made efforts, but the effect was little, Chern on the geometric really high hopes also developed some achievements. But I still and general view on the international consensus, that is the Finsler geometry bleak. This is also the essential reason of Finsler geometry has been unable to enter the mainstream of differential geometry, it no beautiful properties really worth geometers to struggle, also do not have what big application value. Later K- exhibition space, Cartan space will not become mainstream, although they are the extension of Riemannnian geometry, but did not get what the big development.In fact, sometimes the promotion of things to get new content is not much, differential geometry is the same, not the object of study, the more ordinary the better, but should be appropriate to the special good. For example, in Riemann manifold, homogeneous Riemann manifold is more special, beautiful nature, homogeneous Riemann manifolds, symmetric Riemann manifold is more special, so nature more beautiful. This is from the analysis of manifold Lie group action angle.From the point of view of metric, the complex structure is given on the even dimensional Riemann manifold, and the complexmanifold is very elegant. Near complex manifolds are complex manifolds only when the near complex structure is integrable. The complex manifold must be orientable, because it is easy to find that its Jacobian must be nonnegative, whereas the real manifold does not have this property in general. To narrow the scope of the Kahler manifold has more good properties, all complex Submanifolds of Kahler manifolds are Kahler manifolds, and minimal submanifolds (Wirtinger theorem), the beautiful results captured the hearts of many differential geometry and algebraic geometry, because other more general manifolds do not set up this beautiful results. If the first Chern number of a three-dimensional Kahler manifold is zero, the Calabi-Yau manifold can be obtained, which is a very interesting manifold for theoretical physicists. The manifold of mirrors of Calabi-Yau manifolds is also a common subject of differential geometry in algebraic geometry. The popular Hodge structure is a subject of endless appeal.Differential geometry, an endless topic. Just as algebraic geometry requires double - rational equivalence as a luxury, differential geometry requires isometric transformations to be difficult. Taxonomy is an eternal subject of mathematics. In group theory, there are single group classification, multi complex function theory, regional classification, algebraic geometry in the classification of algebraic clusters, differential geometry is also classified.The hard question has led to a dash of young geometry and old scholars, and the prospect of differential geometry is very bright.。
On gradient bounds for the heat kernel on the Heisenberg group

D. Bakry, F. Baudoin, M. Bonnefont, D. Chafa¨ ı
Institut de Math´ ematiques de Toulouse Universit´ e de Toulouse
n
L(f )(x) =
i,j =1
2 f (x). ai,j (x)∂x i xj
The coefficients x → ai,j (x) are smooth and the symmetric matrix (ai,j (x))1≤i,j ≤n is positive definite for every x. The “length of the gradient” |∇f | of a smooth f : M → R is given by Γ(f, f ) = |∇f | =
Contents
1 Introduction 2 Elementary properties of the Heisenberg group 3 Reverse Poincar´ e inequalities 4 A proof of the Driver-Melcher inequality 1 4 7 9
1
Introduction
Gradient bounds had proved to be a very efficient tool for the control of the rate of convergence to equilibrium, quantitative estimates on the regularization properties of heat kernels, functional 1
Empirical processes of dependent random variables

2
Preliminaries
n i=1
from R to R. The centered G -indexed empirical process is given by (P n − P )g = 1 n
n
the marginal and empirical distribution functions. Let G be a class of measurabrocesses that have been discussed include linear processes and Gaussian processes; see Dehling and Taqqu (1989) and Cs¨ org˝ o and Mielniczuk (1996) for long and short-range dependent subordinated Gaussian processes and Ho and Hsing (1996) and Wu (2003a) for long-range dependent linear processes. A collection of recent results is presented in Dehling, Mikosch and Sorensen (2002). In that collection Dedecker and Louhichi (2002) made an important generalization of Ossiander’s (1987) result. Here we investigate the empirical central limit problem for dependent random variables from another angle that avoids strong mixing conditions. In particular, we apply a martingale method and establish a weak convergence theory for stationary, causal processes. Our results are comparable with the theory for independent random variables in that the imposed moment conditions are optimal or almost optimal. We show that, if the process is short-range dependent in a certain sense, then the limiting behavior is similar to that of iid random variables in that the limiting distribution is a Gaussian process and the norming √ sequence is n. For long-range dependent linear processes, one needs to apply asymptotic √ expansions to obtain n-norming limit theorems (Section 6.2.2). The paper is structured as follows. In Section 2 we introduce some mathematical preliminaries necessary for the weak convergence theory and illustrate the essence of our approach. Two types of empirical central limit theorems are established. Empirical processes indexed by indicators of left half lines, absolutely continuous functions, and piecewise differentiable functions are discussed in Sections 3, 4 and 5 respectively. Applications to linear processes and iterated random functions are made in Section 6. Section 7 presents some integral and maximal inequalities that may be of independent interest. Some proofs are given in Sections 8 and 9.
SPSS词汇中英文对照

SPSS词汇(中英文对照) Absolute deviation, 绝对离差Absolute number, 绝对数Absolute residuals, 绝对残差Acceleration array, 加速度立体阵Acceleration in an arbitrary direction, 任意方向上的加速度Acceleration normal, 法向加速度Acceleration space dimension, 加速度空间的维数Acceleration tangential, 切向加速度Acceleration vector, 加速度向量Acceptable hypothesis, 可接受假设Accumulation, 累积Accuracy, 准确度Actual frequency, 实际频数Adaptive estimator, 自适应估计量Addition, 相加Addition theorem, 加法定理Additivity, 可加性Adjusted rate, 调整率Adjusted value, 校正值Admissible error, 容许误差Aggregation, 聚集性Alternative hypothesis, 备择假设Among groups, 组间Amounts, 总量Analysis of correlation, 相关分析Analysis of covariance, 协方差分析Analysis of regression, 回归分析Analysis of time series, 时间序列分析Analysis of variance, 方差分析Angular transformation, 角转换ANOVA (analysis of variance), 方差分析ANOVA Models, 方差分析模型Arcing, 弧/弧旋Arcsine transformation, 反正弦变换Area under the curve, 曲线面积AREG , 评估从一个时间点到下一个时间点回归相关时的误差ARIMA, 季节和非季节性单变量模型的极大似然估计Arithmetic grid paper, 算术格纸Arithmetic mean, 算术平均数Arrhenius relation, 艾恩尼斯关系Assessing fit, 拟合的评估Associative laws, 结合律Asymmetric distribution, 非对称分布Asymptotic bias, 渐近偏倚Asymptotic efficiency, 渐近效率Asymptotic variance, 渐近方差Attributable risk, 归因危险度Attribute data, 属性资料Attribution, 属性Autocorrelation, 自相关Autocorrelation of residuals, 残差的自相关Average, 平均数Average confidence interval length, 平均置信区间长度Average growth rate, 平均增长率Bar chart, 条形图Bar graph, 条形图Base period, 基期Bayes' theorem , Bayes定理Bell-shaped curve, 钟形曲线Bernoulli distribution, 伯努力分布Best-trim estimator, 最好切尾估计量Bias, 偏性Binary logistic regression, 二元逻辑斯蒂回归Binomial distribution, 二项分布Bisquare, 双平方Bivariate Correlate, 二变量相关Bivariate normal distribution, 双变量正态分布Bivariate normal population, 双变量正态总体Biweight interval, 双权区间Biweight M-estimator, 双权M估计量Block, 区组/配伍组BMDP(Biomedical computer programs), BMDP统计软件包Boxplots, 箱线图/箱尾图Breakdown bound, 崩溃界/崩溃点Canonical correlation, 典型相关Caption, 纵标目Case-control study, 病例对照研究Categorical variable, 分类变量Catenary, 悬链线Cauchy distribution, 柯西分布Cause-and-effect relationship, 因果关系Cell, 单元Censoring, 终检Center of symmetry, 对称中心Centering and scaling, 中心化和定标Central tendency, 集中趋势Central value, 中心值CHAID -χ2 Automatic Interaction Detector, 卡方自动交互检测Chance, 机遇Chance error, 随机误差Chance variable, 随机变量Characteristic equation, 特征方程Characteristic root, 特征根Characteristic vector, 特征向量Chebshev criterion of fit, 拟合的切比雪夫准则Chernoff faces, 切尔诺夫脸谱图Chi-square test, 卡方检验/χ2检验Choleskey decomposition, 乔洛斯基分解Circle chart, 圆图Class interval, 组距Class mid-value, 组中值Class upper limit, 组上限Classified variable, 分类变量Cluster analysis, 聚类分析Cluster sampling, 整群抽样Code, 代码Coded data, 编码数据Coding, 编码Coefficient of contingency, 列联系数Coefficient of determination, 决定系数Coefficient of multiple correlation, 多重相关系数Coefficient of partial correlation, 偏相关系数Coefficient of production-moment correlation, 积差相关系数Coefficient of rank correlation, 等级相关系数Coefficient of regression, 回归系数Coefficient of skewness, 偏度系数Coefficient of variation, 变异系数Cohort study, 队列研究Column, 列Column effect, 列效应Column factor, 列因素Combination pool, 合并Combinative table, 组合表Common factor, 共性因子Common regression coefficient, 公共回归系数Common value, 共同值Common variance, 公共方差Common variation, 公共变异Communality variance, 共性方差Comparability, 可比性Comparison of bathes, 批比较Comparison value, 比较值Compartment model, 分部模型Compassion, 伸缩Complement of an event, 补事件Complete association, 完全正相关Complete dissociation, 完全不相关Complete statistics, 完备统计量Completely randomized design, 完全随机化设计Composite event, 联合事件Composite events, 复合事件Concavity, 凹性Conditional expectation, 条件期望Conditional likelihood, 条件似然Conditional probability, 条件概率Conditionally linear, 依条件线性Confidence interval, 置信区间Confidence limit, 置信限Confidence lower limit, 置信下限Confidence upper limit, 置信上限Confirmatory Factor Analysis , 验证性因子分析Confirmatory research, 证实性实验研究Confounding factor, 混杂因素Conjoint, 联合分析Consistency, 相合性Consistency check, 一致性检验Consistent asymptotically normal estimate, 相合渐近正态估计Consistent estimate, 相合估计Constrained nonlinear regression, 受约束非线性回归Constraint, 约束Contaminated distribution, 污染分布Contaminated Gausssian, 污染高斯分布Contaminated normal distribution, 污染正态分布Contamination, 污染Contamination model, 污染模型Contingency table, 列联表Contour, 边界线Contribution rate, 贡献率Control, 对照Controlled experiments, 对照实验Conventional depth, 常规深度Convolution, 卷积Corrected factor, 校正因子Corrected mean, 校正均值Correction coefficient, 校正系数Correctness, 正确性Correlation coefficient, 相关系数Correlation index, 相关指数Correspondence, 对应Counting, 计数Counts, 计数/频数Covariance, 协方差Covariant, 共变Cox Regression, Cox回归Criteria for fitting, 拟合准则Criteria of least squares, 最小二乘准则Critical ratio, 临界比Critical region, 拒绝域Critical value, 临界值Cross-over design, 交叉设计Cross-section analysis, 横断面分析Cross-section survey, 横断面调查Crosstabs , 交叉表Cross-tabulation table, 复合表Cube root, 立方根Cumulative distribution function, 分布函数Cumulative probability, 累计概率Curvature, 曲率/弯曲Curvature, 曲率Curve fit , 曲线拟和Curve fitting, 曲线拟合Curvilinear regression, 曲线回归Curvilinear relation, 曲线关系Cut-and-try method, 尝试法Cycle, 周期Cyclist, 周期性D test, D检验Data acquisition, 资料收集Data bank, 数据库Data capacity, 数据容量Data deficiencies, 数据缺乏Data handling, 数据处理Data manipulation, 数据处理Data processing, 数据处理Data reduction, 数据缩减Data set, 数据集Data sources, 数据来源Data transformation, 数据变换Data validity, 数据有效性Data-in, 数据输入Data-out, 数据输出Dead time, 停滞期Degree of freedom, 自由度Degree of precision, 精密度Degree of reliability, 可靠性程度Degression, 递减Density function, 密度函数Density of data points, 数据点的密度Dependent variable, 应变量/依变量/因变量Dependent variable, 因变量Depth, 深度Derivative matrix, 导数矩阵Derivative-free methods, 无导数方法Design, 设计Determinacy, 确定性Determinant, 行列式Determinant, 决定因素Deviation, 离差Deviation from average, 离均差Diagnostic plot, 诊断图Dichotomous variable, 二分变量Differential equation, 微分方程Direct standardization, 直接标准化法Discrete variable, 离散型变量DISCRIMINANT, 判断Discriminant analysis, 判别分析Discriminant coefficient, 判别系数Discriminant function, 判别值Dispersion, 散布/分散度Disproportional, 不成比例的Disproportionate sub-class numbers, 不成比例次级组含量Distribution free, 分布无关性/免分布Distribution shape, 分布形状Distribution-free method, 任意分布法Distributive laws, 分配律Disturbance, 随机扰动项Dose response curve, 剂量反应曲线Double blind method, 双盲法Double blind trial, 双盲试验Double exponential distribution, 双指数分布Double logarithmic, 双对数Downward rank, 降秩Dual-space plot, 对偶空间图DUD, 无导数方法Duncan's new multiple range method, 新复极差法/Duncan新法Effect, 实验效应Eigenvalue, 特征值Eigenvector, 特征向量Ellipse, 椭圆Empirical distribution, 经验分布Empirical probability, 经验概率单位Enumeration data, 计数资料Equal sun-class number, 相等次级组含量Equally likely, 等可能Equivariance, 同变性Error, 误差/错误Error of estimate, 估计误差Error type I, 第一类错误Error type II, 第二类错误Estimand, 被估量Estimated error mean squares, 估计误差均方Estimated error sum of squares, 估计误差平方和Euclidean distance, 欧式距离Event, 事件Event, 事件Exceptional data point, 异常数据点Expectation plane, 期望平面Expectation surface, 期望曲面Expected values, 期望值Experiment, 实验Experimental sampling, 试验抽样Experimental unit, 试验单位Explanatory variable, 说明变量Exploratory data analysis, 探索性数据分析Explore Summarize, 探索-摘要Exponential curve, 指数曲线Exponential growth, 指数式增长EXSMOOTH, 指数平滑方法Extended fit, 扩充拟合Extra parameter, 附加参数Extrapolation, 外推法Extreme observation, 末端观测值Extremes, 极端值/极值F distribution, F分布F test, F检验Factor, 因素/因子Factor analysis, 因子分析Factor Analysis, 因子分析Factor score, 因子得分Factorial, 阶乘Factorial design, 析因试验设计False negative, 假阴性False negative error, 假阴性错误Family of distributions, 分布族Family of estimators, 估计量族Fanning, 扇面Fatality rate, 病死率Field investigation, 现场调查Field survey, 现场调查Finite population, 有限总体Finite-sample, 有限样本First derivative, 一阶导数First principal component, 第一主成分First quartile, 第一四分位数Fisher information, 费雪信息量Fitted value, 拟合值Fitting a curve, 曲线拟合Fixed base, 定基Fluctuation, 随机起伏Forecast, 预测Four fold table, 四格表Fourth, 四分点Fraction blow, 左侧比率Fractional error, 相对误差Frequency, 频率Frequency polygon, 频数多边图Frontier point, 界限点Function relationship, 泛函关系Gamma distribution, 伽玛分布Gauss increment, 高斯增量Gaussian distribution, 高斯分布/正态分布Gauss-Newton increment, 高斯-牛顿增量General census, 全面普查GENLOG (Generalized liner models), 广义线性模型Geometric mean, 几何平均数Gini's mean difference, 基尼均差GLM (General liner models), 一般线性模型Goodness of fit, 拟和优度/配合度Gradient of determinant, 行列式的梯度Graeco-Latin square, 希腊拉丁方Grand mean, 总均值Gross errors, 重大错误Gross-error sensitivity, 大错敏感度Group averages, 分组平均Grouped data, 分组资料Guessed mean, 假定平均数Half-life, 半衰期Hampel M-estimators, 汉佩尔M估计量Happenstance, 偶然事件Harmonic mean, 调和均数Hazard function, 风险均数Hazard rate, 风险率Heading, 标目Heavy-tailed distribution, 重尾分布Hessian array, 海森立体阵Heterogeneity, 不同质Heterogeneity of variance, 方差不齐Hierarchical classification, 组内分组Hierarchical clustering method, 系统聚类法High-leverage point, 高杠杆率点HILOGLINEAR, 多维列联表的层次对数线性模型Hinge, 折叶点Histogram, 直方图Historical cohort study, 历史性队列研究Holes, 空洞HOMALS, 多重响应分析Homogeneity of variance, 方差齐性Homogeneity test, 齐性检验Huber M-estimators, 休伯M估计量Hyperbola, 双曲线Hypothesis testing, 假设检验Hypothetical universe, 假设总体Impossible event, 不可能事件Independence, 独立性Independent variable, 自变量Index, 指标/指数Indirect standardization, 间接标准化法Individual, 个体Inference band, 推断带Infinite population, 无限总体Infinitely great, 无穷大Infinitely small, 无穷小Influence curve, 影响曲线Information capacity, 信息容量Initial condition, 初始条件Initial estimate, 初始估计值Initial level, 最初水平Interaction, 交互作用Interaction terms, 交互作用项Intercept, 截距Interpolation, 内插法Interquartile range, 四分位距Interval estimation, 区间估计Intervals of equal probability, 等概率区间Intrinsic curvature, 固有曲率Invariance, 不变性Inverse matrix, 逆矩阵Inverse probability, 逆概率Inverse sine transformation, 反正弦变换Iteration, 迭代Jacobian determinant, 雅可比行列式Joint distribution function, 分布函数Joint probability, 联合概率Joint probability distribution, 联合概率分布K means method, 逐步聚类法Kaplan-Meier, 评估事件的时间长度Kaplan-Merier chart, Kaplan-Merier图Kendall's rank correlation, Kendall等级相关Kinetic, 动力学Kolmogorov-Smirnove test, 柯尔莫哥洛夫-斯米尔诺夫检验Kruskal and Wallis test, Kruskal及Wallis检验/多样本的秩和检验/H检验Kurtosis, 峰度Lack of fit, 失拟Ladder of powers, 幂阶梯Lag, 滞后Large sample, 大样本Large sample test, 大样本检验Latin square, 拉丁方Latin square design, 拉丁方设计Leakage, 泄漏Least favorable configuration, 最不利构形Least favorable distribution, 最不利分布Least significant difference, 最小显著差法Least square method, 最小二乘法Least-absolute-residuals estimates, 最小绝对残差估计Least-absolute-residuals fit, 最小绝对残差拟合Least-absolute-residuals line, 最小绝对残差线Legend, 图例L-estimator, L估计量L-estimator of location, 位置L估计量L-estimator of scale, 尺度L估计量Level, 水平Life expectance, 预期期望寿命Life table, 寿命表Life table method, 生命表法Light-tailed distribution, 轻尾分布Likelihood function, 似然函数Likelihood ratio, 似然比line graph, 线图Linear correlation, 直线相关Linear equation, 线性方程Linear programming, 线性规划Linear regression, 直线回归Linear Regression, 线性回归Linear trend, 线性趋势Loading, 载荷Location and scale equivariance, 位置尺度同变性Location equivariance, 位置同变性Location invariance, 位置不变性Location scale family, 位置尺度族Log rank test, 时序检验Logarithmic curve, 对数曲线Logarithmic normal distribution, 对数正态分布Logarithmic scale, 对数尺度Logarithmic transformation, 对数变换Logic check, 逻辑检查Logistic distribution, 逻辑斯特分布Logit transformation, Logit转换LOGLINEAR, 多维列联表通用模型Lognormal distribution, 对数正态分布Lost function, 损失函数Low correlation, 低度相关Lower limit, 下限Lowest-attained variance, 最小可达方差LSD, 最小显著差法的简称Lurking variable, 潜在变量Main effect, 主效应Major heading, 主辞标目Marginal density function, 边缘密度函数Marginal probability, 边缘概率Marginal probability distribution, 边缘概率分布Matched data, 配对资料Matched distribution, 匹配过分布Matching of distribution, 分布的匹配Matching of transformation, 变换的匹配Mathematical expectation, 数学期望Mathematical model, 数学模型Maximum L-estimator, 极大极小L 估计量Maximum likelihood method, 最大似然法Mean, 均数Mean squares between groups, 组间均方Mean squares within group, 组内均方Means (Compare means), 均值-均值比较Median, 中位数Median effective dose, 半数效量Median lethal dose, 半数致死量Median polish, 中位数平滑Median test, 中位数检验Minimal sufficient statistic, 最小充分统计量Minimum distance estimation, 最小距离估计Minimum effective dose, 最小有效量Minimum lethal dose, 最小致死量Minimum variance estimator, 最小方差估计量MINITAB, 统计软件包Minor heading, 宾词标目Missing data, 缺失值Model specification, 模型的确定Modeling Statistics , 模型统计Models for outliers, 离群值模型Modifying the model, 模型的修正Modulus of continuity, 连续性模Morbidity, 发病率Most favorable configuration, 最有利构形Multidimensional Scaling (ASCAL), 多维尺度/多维标度Multinomial Logistic Regression , 多项逻辑斯蒂回归Multiple comparison, 多重比较Multiple correlation , 复相关Multiple covariance, 多元协方差Multiple linear regression, 多元线性回归Multiple response , 多重选项Multiple solutions, 多解Multiplication theorem, 乘法定理Multiresponse, 多元响应Multi-stage sampling, 多阶段抽样Multivariate T distribution, 多元T分布Mutual exclusive, 互不相容Mutual independence, 互相独立Natural boundary, 自然边界Natural dead, 自然死亡Natural zero, 自然零Negative correlation, 负相关Negative linear correlation, 负线性相关Negatively skewed, 负偏Newman-Keuls method, q检验NK method, q检验No statistical significance, 无统计意义Nominal variable, 名义变量Nonconstancy of variability, 变异的非定常性Nonlinear regression, 非线性相关Nonparametric statistics, 非参数统计Nonparametric test, 非参数检验Nonparametric tests, 非参数检验Normal deviate, 正态离差Normal distribution, 正态分布Normal equation, 正规方程组Normal ranges, 正常范围Normal value, 正常值Nuisance parameter, 多余参数/讨厌参数Null hypothesis, 无效假设Numerical variable, 数值变量Objective function, 目标函数Observation unit, 观察单位Observed value, 观察值One sided test, 单侧检验One-way analysis of variance, 单因素方差分析Oneway ANOVA , 单因素方差分析Open sequential trial, 开放型序贯设计Optrim, 优切尾Optrim efficiency, 优切尾效率Order statistics, 顺序统计量Ordered categories, 有序分类Ordinal logistic regression , 序数逻辑斯蒂回归Ordinal variable, 有序变量Orthogonal basis, 正交基Orthogonal design, 正交试验设计Orthogonality conditions, 正交条件ORTHOPLAN, 正交设计Outlier cutoffs, 离群值截断点Outliers, 极端值OVERALS , 多组变量的非线性正规相关Overshoot, 迭代过度Paired design, 配对设计Paired sample, 配对样本Pairwise slopes, 成对斜率Parabola, 抛物线Parallel tests, 平行试验Parameter, 参数Parametric statistics, 参数统计Parametric test, 参数检验Partial correlation, 偏相关Partial regression, 偏回归Partial sorting, 偏排序Partials residuals, 偏残差Pattern, 模式Pearson curves, 皮尔逊曲线Peeling, 退层Percent bar graph, 百分条形图Percentage, 百分比Percentile, 百分位数Percentile curves, 百分位曲线Periodicity, 周期性Permutation, 排列P-estimator, P估计量Pie graph, 饼图Pitman estimator, 皮特曼估计量Pivot, 枢轴量Planar, 平坦Planar assumption, 平面的假设PLANCARDS, 生成试验的计划卡Point estimation, 点估计Poisson distribution, 泊松分布Polishing, 平滑Polled standard deviation, 合并标准差Polled variance, 合并方差Polygon, 多边图Polynomial, 多项式Polynomial curve, 多项式曲线Population, 总体Population attributable risk, 人群归因危险度Positive correlation, 正相关Positively skewed, 正偏Posterior distribution, 后验分布Power of a test, 检验效能Precision, 精密度Predicted value, 预测值Preliminary analysis, 预备性分析Principal component analysis, 主成分分析Prior distribution, 先验分布Prior probability, 先验概率Probabilistic model, 概率模型probability, 概率Probability density, 概率密度Product moment, 乘积矩/协方差Profile trace, 截面迹图Proportion, 比/构成比Proportion allocation in stratified random sampling, 按比例分层随机抽样Proportionate, 成比例Proportionate sub-class numbers, 成比例次级组含量Prospective study, 前瞻性调查Proximities, 亲近性Pseudo F test, 近似F检验Pseudo model, 近似模型Pseudosigma, 伪标准差Purposive sampling, 有目的抽样QR decomposition, QR分解Quadratic approximation, 二次近似Qualitative classification, 属性分类Qualitative method, 定性方法Quantile-quantile plot, 分位数-分位数图/Q-Q图Quantitative analysis, 定量分析Quartile, 四分位数Quick Cluster, 快速聚类Radix sort, 基数排序Random allocation, 随机化分组Random blocks design, 随机区组设计Random event, 随机事件Randomization, 随机化Range, 极差/全距Rank correlation, 等级相关Rank sum test, 秩和检验Rank test, 秩检验Ranked data, 等级资料Rate, 比率Ratio, 比例Raw data, 原始资料Raw residual, 原始残差Rayleigh's test, 雷氏检验Rayleigh's Z, 雷氏Z值Reciprocal, 倒数Reciprocal transformation, 倒数变换Recording, 记录Redescending estimators, 回降估计量Reducing dimensions, 降维Re-expression, 重新表达Reference set, 标准组Region of acceptance, 接受域Regression coefficient, 回归系数Regression sum of square, 回归平方和Rejection point, 拒绝点Relative dispersion, 相对离散度Relative number, 相对数Reliability, 可靠性Reparametrization, 重新设置参数Replication, 重复Report Summaries, 报告摘要Residual sum of square, 剩余平方和Resistance, 耐抗性Resistant line, 耐抗线Resistant technique, 耐抗技术R-estimator of location, 位置R估计量R-estimator of scale, 尺度R估计量Retrospective study, 回顾性调查Ridge trace, 岭迹Ridit analysis, Ridit分析Rotation, 旋转Rounding, 舍入Row, 行Row effects, 行效应Row factor, 行因素RXC table, RXC表Sample, 样本Sample regression coefficient, 样本回归系数Sample size, 样本量Sample standard deviation, 样本标准差Sampling error, 抽样误差SAS(Statistical analysis system ), SAS统计软件包Scale, 尺度/量表Scatter diagram, 散点图Schematic plot, 示意图/简图Score test, 计分检验Screening, 筛检SEASON, 季节分析Second derivative, 二阶导数Second principal component, 第二主成分SEM (Structural equation modeling), 结构化方程模型Semi-logarithmic graph, 半对数图Semi-logarithmic paper, 半对数格纸Sensitivity curve, 敏感度曲线Sequential analysis, 贯序分析Sequential data set, 顺序数据集Sequential design, 贯序设计Sequential method, 贯序法Sequential test, 贯序检验法Serial tests, 系列试验Short-cut method, 简捷法Sigmoid curve, S形曲线Sign function, 正负号函数Sign test, 符号检验Signed rank, 符号秩Significance test, 显著性检验Significant figure, 有效数字Simple cluster sampling, 简单整群抽样Simple correlation, 简单相关Simple random sampling, 简单随机抽样Simple regression, 简单回归simple table, 简单表Sine estimator, 正弦估计量Single-valued estimate, 单值估计Singular matrix, 奇异矩阵Skewed distribution, 偏斜分布Skewness, 偏度Slash distribution, 斜线分布Slope, 斜率Smirnov test, 斯米尔诺夫检验Source of variation, 变异来源Spearman rank correlation, 斯皮尔曼等级相关Specific factor, 特殊因子Specific factor variance, 特殊因子方差Spectra , 频谱Spherical distribution, 球型正态分布Spread, 展布SPSS(Statistical package for the social science), SPSS统计软件包Spurious correlation, 假性相关Square root transformation, 平方根变换Stabilizing variance, 稳定方差Standard deviation, 标准差Standard error, 标准误Standard error of difference, 差别的标准误Standard error of estimate, 标准估计误差Standard error of rate, 率的标准误Standard normal distribution, 标准正态分布Standardization, 标准化Starting value, 起始值Statistic, 统计量Statistical control, 统计控制Statistical graph, 统计图Statistical inference, 统计推断Statistical table, 统计表Steepest descent, 最速下降法Stem and leaf display, 茎叶图Step factor, 步长因子Stepwise regression, 逐步回归Storage, 存Strata, 层(复数)Stratified sampling, 分层抽样Stratified sampling, 分层抽样Strength, 强度Stringency, 严密性Structural relationship, 结构关系Studentized residual, 学生化残差/t化残差Sub-class numbers, 次级组含量Subdividing, 分割Sufficient statistic, 充分统计量Sum of products, 积和Sum of squares, 离差平方和Sum of squares about regression, 回归平方和Sum of squares between groups, 组间平方和Sum of squares of partial regression, 偏回归平方和Sure event, 必然事件Survey, 调查Survival, 生存分析Survival rate, 生存率Suspended root gram, 悬吊根图Symmetry, 对称Systematic error, 系统误差Systematic sampling, 系统抽样Tags, 标签Tail area, 尾部面积Tail length, 尾长Tail weight, 尾重Tangent line, 切线Target distribution, 目标分布Taylor series, 泰勒级数Tendency of dispersion, 离散趋势Testing of hypotheses, 假设检验Theoretical frequency, 理论频数Time series, 时间序列Tolerance interval, 容忍区间Tolerance lower limit, 容忍下限Tolerance upper limit, 容忍上限Torsion, 扰率Total sum of square, 总平方和Total variation, 总变异Transformation, 转换Treatment, 处理Trend, 趋势Trend of percentage, 百分比趋势Trial, 试验Trial and error method, 试错法Tuning constant, 细调常数Two sided test, 双向检验Two-stage least squares, 二阶最小平方Two-stage sampling, 二阶段抽样Two-tailed test, 双侧检验Two-way analysis of variance, 双因素方差分析Two-way table, 双向表Type I error, 一类错误/α错误Type II error, 二类错误/β错误UMVU, 方差一致最小无偏估计简称Unbiased estimate, 无偏估计Unconstrained nonlinear regression , 无约束非线性回归Unequal subclass number, 不等次级组含量Ungrouped data, 不分组资料Uniform coordinate, 均匀坐标Uniform distribution, 均匀分布Uniformly minimum variance unbiased estimate, 方差一致最小无偏估计Unit, 单元Unordered categories, 无序分类Upper limit, 上限Upward rank, 升秩Vague concept, 模糊概念Validity, 有效性VARCOMP (Variance component estimation), 方差元素估计Variability, 变异性Variable, 变量Variance, 方差Variation, 变异Varimax orthogonal rotation, 方差最大正交旋转Volume of distribution, 容积W test, W检验Weibull distribution, 威布尔分布Weight, 权数Weighted Chi-square test, 加权卡方检验/Cochran检验Weighted linear regression method, 加权直线回归Weighted mean, 加权平均数Weighted mean square, 加权平均方差Weighted sum of square, 加权平方和Weighting coefficient, 权重系数Weighting method, 加权法W-estimation, W估计量W-estimation of location, 位置W估计量Width, 宽度Wilcoxon paired test, 威斯康星配对法/配对符号秩和检验Wild point, 野点/狂点Wild value, 野值/狂值Winsorized mean, 缩尾均值Withdraw, 失访Youden's index, 尤登指数Z test, Z检验Zero correlation, 零相关Z-transformation, Z变换。
Triangulations of Seifert Fibred Manifolds

a r X i v :m a t h /0301246v 1 [m a t h .G T ] 22 J a n 2003TRIANGULATIONS OF SEIFERT FIBRED MANIFOLDSALEKSANDAR MIJATOVI ´CAbstract .It is not completely unreasonable to expect that a computable function bounding the number of Pachner moves needed to change any triangulation of a given 3-manifold into any other triangulation of the same 3-manifold exists.In this paper we describe a procedure yielding an explicit formula for such a function if the 3-manifold in question is a Seifert fibred space.1INTRODUCTION There is a natural way of modifying a triangulation T of an n -manifold.Suppose D is a combinatorial n -disc which is a subcomplex both in this triangulation and in the boundary of the (n +1)-simplex ∆n +1.We can change T by removing D and inserting ∆n +1−int(D ).What we’ve just described is called a Pachner move .In dimension 3there are four possible moves (see figure 1).Note that we can define Pachner moves even if the triangulation T is non-combinatorial (i.e.simplices of T are not uniquely determined by their vertices).Since our aim is to deal with the triangulations of the manifolds that are not necessarily closed,we need to allow for some additional moves that will modify the simplicial structure on the boundary (throughout this paper we will be using the term simplicial structure as a synonym for a possibly non-combinatorial triangulation).The definition of a Pachner move readily generalises to this setting.Changing the triangulation of the boundary by an (n −1)-dimensional Pachner move amounts to gluing onto (or removing form)our manifold an n -simplex ∆n ,which must exist by the definition of the move.So in dimension 3we have to use the three two-dimensional moves (usually referred to as (2−2),(1−3)and (3−1))that can be implemented by gluing on or shelling a tetrahedron.It was proved by Pachner (see [9])that any two triangulations of the same PL n -manifold are related by a finite sequence of Pachner moves and simplicial isomorphisms.It is well known (see proposition 1.3in [8])that a computable function bounding the length of the sequence from Pach-ner’s theorem in terms of the number of tetrahedra for a fixed 3-manifold M ,gives an algorithm for recognising M among all 3-manifolds.The following theorem gives an explicit formula for such a bound in case M is a Seifert fibred space with a fixed triangulation on its boundary.Theorem 1.1Let M →B be a Seifert fibred space with non-empty boundary.Let P and Q be two triangulations of M that coincide on ∂M and contain p and q tetrahedra respectively.Then there exists a sequence of Pachner moves of length at most e 6(10p )+e 6(10q )which transforms P into a triangulation isomorphic to Q .The homeomorphism of M that realizes the simplicial isomorphismis,when restricted to ∂M ,equal to the identity on the boundary of M .The exponent in the above expression containing the exponential function e (x )=2x stands for the composition of the function with itself rather than for multiplication.The shameful enormity of the bound can be curbed by a more careful choice of subdivisions.The height of the tower of exponents can be reduced from 6to 3,but the complexity of the constructions involved grows tenfold.Since we are mainly interested in the existence of an explicit formula,we shall not strive to get the best numbers possible.The bound in theorem 1.1is clearly computable and it hence gives a conceptually simple recognition algorithm for every bounded Seifert fibred space.It is true that the topology of1(2-3)(1-4)(4-1)Figure1:Three dimensional Pachner moves.the Seifertfibred manifolds is not terribly exciting.They are however ubiquitous pieces in JSJ-decompositions of more interesting3-manifolds.It is there that our theorem willfind its best application.2SOME NORMAL SURF ACE THEORY AND SOME TOPOLOGY The pivotal tool for probing the triangulations of our3-manifolds is normal surface theory. Many good accounts of it appeared in the literature(see for example[2],[6]or[1]).In this section we will state the basic definitions that will allow us to give some known properties of normal surface.We will then go on to explore how normal surfaces interact with boundary patterns.The section will be concluded with a discussion of some well known features of incompressible surfaces in Seifertfibred manifolds.We are assuming throughout that all3-manifolds we are dealing with are orientable.Let T be a triangulation of a3-manifold M.An arc in a2-simplex of T is normal if its ends lie in different sides of a2-simplex.A simple closed curve in the2-skeleton of T is a normal curve if it intersects each2-simplex of T in normal arcs.A properly embedded surface F in M is in normal form with respect to T if it intersects each tetrahedron in T in a collection of discs all of whose boundaries are normal curves consisting of3or4normal arcs,i.e.triangles and quadrilaterals.A normal discs is a triangle or a quadrilateral.There are precisely seven normal disc types in any tetrahedron of T.An isotopy of M is called a normal isotopy with respect to T if it leaves all simplices of T invariant.In particular this implies that it isfixed on the vertices of T.A normal surface is determined,up to normal isotopy,by the number of normal disc types in which it meets the tetrahedra of T.It therefore defines a vector with7t coordinates.Each coordinate represents the number of copies of a normal disc type that are contained in the surface with t being the number of tetrahedra in T.It turns out that there is a certain restricted linear system that such a vector is a solution of.Moreover there is a one to one correspondence between the solutions of that restricted linear system and normal surface in M.If the sum of two vector solutions of this system satisfies the restrictions on the system,then it represents a normal surface in M.On the other hand there is a geometric process called regular alteration(seefigure2in[1]) which can be carried out on the normal surfaces representing the summands and which yields the2normal surface corresponding to the sum.It follows directly from the definition of regular alteration that the Euler characteristic is additive over normal addition.We can define the weight w(F)of a surface F,which is transverse to the1-skeleton of T,to be the number of points of intersection between the surface and the1-skeleton.Since regular alteration only changes the surfaces involved away from the1-skeleton,the weight too is additive over normal addition.A normal surface is called fundamental if the vector corresponding to it is not a sum of two integral solutions of the linear system.The solution space of the linear system projects down to a compact convex linear cell which is called the projective solution space.A vertex surface is a connected two-sided normal surface that projects onto a vertex of the projective solution space (see[6]for a more detailed description).The next proposition is proved in[2].It will be important for us that its proof does not de-pend on the number of equations in the linear system arising from the triangulation of the manifold. Proposition2.1Let M be a compact triangulated3-manifold containing t tetrahedra.Then each normal coordinate of a vertex surface in M is bounded above by27t.If the normal surface is fundamental,then7t27t puts an upper bound on all of its normal coordinates.Recall that a properly embedded surface F in a3-manifold M is injective if the homomorphism π1(F)→π1(M),induced by the inclusion of F into M,is a monomorphism.A surface F is said to be incompressible if it satisfies the following conditions:•The surface F does not contain2-spheres that bound3-balls nor does it contain discs which are isotopic rel boundary to discs in∂M and•for every disc D in M with D∩S=∂D there is a disc D′in S with∂D=∂D′.A horizontal boundary of an I-bundle over a surface is a part of the boundary corresponding to the∂I-bundle.The vertical boundary is a complement of the horizontal boundary and consists of annuli thatfibre over the bounding circles of the base surface.It is a well-known fact that a properly embedded one-sided surface in M is injective if and only if the horizontal boundary of its regular neighbourhood is incompressible.An embedded torus in M that is incompressible and is not boundary parallel is sometimes referred to as an essential torus.There is also a relative notion of incompressibility which we will need to consider.A surface F is∂-incompressible if for each disc D in M such that∂D splits into two arcsαandβmeeting only at their common endpoints,with D∩F=αand D∩∂M=βthere is a disc D′in F with α⊂∂D′and∂D′−α⊂∂F.Such a disc D is called a∂-compression disc for F.Note that if the manifold M is irreducible and has incompressible boundary,then we can isotope F relαso that the disc D′becomes the∂-compression disc D.A properly embedded annulus in M that is both incompressible and∂-incompressible is called an essential annulus.Before we can state the main technical results from normal surface theory,we need to define a very useful concept.Definition.A boundary pattern P in a compact3-manifold M is a(possibly empty)collection of disjoint simple closed curves and trivalent graphs embedded in∂M such that the surface∂M−P is incompressible in M.Boundary patterns usually appear in the context of hierarchies.In this paper however we will mainly be concerned with a special case when the pattern P consists of simple closed curves only.Therefore we will not investigate the correspondence between hierarchies and patterns any further.Notice also that it follows from the definition of the pattern that any simple closed curve component of P is homotopically non-trivial in∂M.Let M be a3-manifold with non-empty boundary that contains a boundary pattern P.It follows from the definition that the pattern P can be empty if and only if the manifold M has incompressible boundary.Assume now that the pattern P is not empty.A subset of M is pure if it has empty intersection with the pattern P.Most concepts from general3-manifolds carry over to3-manifolds with pattern in a very natural way.For example,a properly embedded surface F in3-manifold M with pattern P is P-boundary incompressible if for any pure disc D in M,such that D∩(∂M∪F)=∂D and D∩F is a single arc in F,the arc D∩F cuts offa pure disc from F.3Notice that for P=∅this notion reduces to∂-incompressibility.Also this notion is well defined only up to an isotopy of M that is invariant on the pattern P.In other words we can have two isotopic surfaces in M,out of which only one is P-boundary incompressible.Let T be some triangulation of a3-manifold M with pattern P.Throughout this paper we will be assuming that the pattern P lies in the1-skeleton of T.This assumption immediately implies that any incompressible P-boundary incompressible surface in M can be isotoped into normal form. Also any normal surface F in M has a well defined intersection numberι(F),equal to the number of points in∂F∩P.Moreover this intersection number is additive over geometric sums of normal surfaces.We say that a surface F in a3-manifold M with pattern P has minimal weight if it can not be isotoped to a surface with lower weight by an isotopy that is invariant on the pattern.In case of P=∅this reduces to the usual definition of a minimal weight.An incompressible P-boundary incompressible surface in M of minimal weight has to intersect each triangle in the2-skeleton of T in normal arcs and possibly some simple closed curves.If M is irreducible we can remove these simple closed curves by isotopies in the usual way.The isotoped surface is then in normal form.The sum F=F1+F2is in reduced form if the number of components of F1∩F2is minimal among all normal surfaces F′1and F′2isotopic rel P to F1and F2respectively such that F=F′1+F′2.If the pattern P is empty we get the familiar notion of reduced form which was used in[1].We are now going to define a concept which will be of some significance to all that follows.Definition.A patch for the normal sum F=F1+F2is a component of F1−int(N(F1∩F2)) or F2−int(N(F1∩F2)).A trivial patch is a pure patch which is topologically a disc and whose boundary intersects only one component of the1-manifold F1∩F2.This means that a boundary of a trivial patch is either a single simple closed curve in F1∩F2or it consists of two arcs:one in(∂M)−P and the other in F1∩F2.Thefirst possibility coincides with what was called a disc-patch in[1].In the absence of pattern the notion of the trivial patch coincides with what is called a disc-patch in[6].The reason for our slightly non-standard terminology is to avoid the confusion arising from the patches which are discs but are not disc-patches.There are several ways in which a disc patch(i.e.a patch which is a disc)can fail to be trivial,if∂M is not empty.Clearly a disc patch which intersects the pattern P is non-trivial.A pure disc patch will also be non-trivial if it intersects∂M in more than one arc.It is not hard to prove that a trivial patch can not have zero weight.The argument of lemma 3.3in[1]gives it to us for all trivial patches bounded by simple closed curve components of F1∩F2. In case when our disc patch is bounded by two arcs,we can use a simple doubling trick and then apply lemma3.3from[1]to obtain the desired conclusion.However there are patches that are topologically discs and have zero weight.But they must contain more than one component of F1∩F2in their boundaries.Now we arefinally in the position to state the following lemma. Lemma2.2Let M be an irreducible3-manifold with a(possibly empty)boundary pattern P.Let F be a minimal weight incompressible P-boundary incompressible normal surface.If the sum F= F1+F2is in reduced form then each patch is both incompressible and P-boundary incompressible and no patch is trivial.Furthermore if F is injective,then each patch has to be injective.This lemma is a mild generalisation of both lemma3.6in[1]and lemma6.6in[6].Even though patches are not properly embedded surfaces in M the notions of P-boundary incompressibility and injectivity can be naturally extended to this setting.Note also that if P=∅,the manifold M has incompressible boundary,the surface F is boundary incompressible and so are the patches of F=F1+F2.Proof.We start by reducing the lemma to the statement that no patch of F1+F2is trivial.In case we have a patch which is either compressible or not injective,we can argue in precisely the same way as in the proof of lemma3.6of[1]to obtain a disc patch whose boundary is a single simple closed curve from F1∩F2and is therefore trivial.If there is a patch R of F1+F2which has a pure boundary compressing disc D,then we can assume without loss of generality that D is also a boundary compressing disc for the surface F.4Then the unique arc D∩R=D∩F cuts offa pure disc D′in F.If D′contains a simple closed curve of F1∩F2,then we canfind a compressible patch of F1+F2and we are in the previous case. If there are no simple closed curves of F1∩F2in D′,then the edge most arc from F1∩F2in D′cuts offa pure disc patch which is clearly trivial.So it is enough to prove that no trivial patch exists.If there exists a disc patch in F1+F2bounded by a single simple closed curve from F1∩F2,we can use an identical argument to the one in the proof of lemma3.6in[1]to construct two normal surfaces F′and S such that F=F′+S.F′is isotopic to F and S is a closed normal surface withχ(S)=w(S)=0.This is clearly a contradiction because no normal surface can miss the 1-skeleton.Also by our hypothesis the surface F might be only incompressible and not necessarily injective.So in order to use the argument from[1]which shows that every simple closed curve in F1∩F2,bounding a trivial patch,has to be two-sided in both F1and F2,we need to note that when M equals R P3,every embedded projective plane in M is actually injective.So now we can assume that F1+F2contains no disc patch disjoint from∂M.Let D be a trivial patch,lying in F1say,which has least weight among all trivial patches in F1+F2.The intersection D∩F2consists of a unique arcαthat is a component of F1∩F2.After regular alterationαproduces two properly embedded arcs in F one of which cuts offa pure disc D′from F.This is because F is P-boundary incompressible.Disc D′is distinct from but might contain the trivial patch D.We must have w(D′)=w(D).Otherwise we could isotope F,by an isotopy invariant on the pattern,so that its weight is decreased.Since D minimises the weight of all trivial patches(which is strictly positive)and D′must contain at least one such,there is exactly one trivial patch in D′and its weight is equal to w(D).Every other patch in D′is topologically a disc whose boundary consists of4arcs.Two of them are in∂M and the other two are components of F1∩F2.If D′was itself a patch then we could isotope F1and F2,using the definition of the pattern P and the irreducibility of M,to obtain normal surfaces F′1and F′2still summing up to F but having fewer components of intersection.This contradicts our assumption on the reduced form of F1+F2.So D′is not itself a trivial patch,but it has to contain one.Now we can imitate the argument in the proof of lemma3.6from[1]to obtain a normal sum F=A+F′where F′is a normal surface isotopic to F and A is a pure normal annulus of zero weight.Since no normal surface can live in the complement of the1-skeleton,this gives afinal contradiction.2 Another crucial fact from normal surface theory,tying up normal addition with the topological properties of surfaces involved,is contained in the next theorem.It appeared several times in the literature in slightly different forms.The version that is of interest to us is the following.Theorem2.3Let M be an irreducible3-manifold with a possibly empty boundary pattern P.Let F be a least weight normal surface properly embedded in M.Assume also that F is two-sided incompressible P-boundary incompressible and F=F1+F2.Then F1and F2are incompressible and P-boundary incompressible.The proof of this theorem can be obtained by using lemma2.2and following(verbatim)the proof of theorem6.5in[6].Before we state a further consequence of theorem2.3,we need the following simple fact from topology.Lemma2.4Let M be an irreducible3-manifold with incompressible boundary.Assume also that M is neither homeomorphic to the product S1×S1×I,nor to an I-bundle over a Klein bottle. Let S be a toral boundary component of M.If A and B are two properly embedded incompressible ∂-incompressible annuli in M such that at least one boundary component of both annuli lies in S, then these bounding simple closed curves must be isotopic in S,i.e.they determine the same slope in S.Proof.We start by isotoping the annuli A and B so that their intersection is minimal.If A∩B is either empty or it consists only of essential simple closed curves,then the boundary curves must5be parallel in S.So we can assume that∂A∩∂B is non-empty.This implies that the boundary slopes of∂A and∂B in S are distinct.Therefore the complement S−(∂A∪∂B)is a disjoint union of disc.Since both A and B are incompressible and∂-incompressible,the intersection contains neither contractible simple closed curves nor boundary parallel arcs in either of the two annuli.In other words A∩B consists of spanning arcs in both annuli.So an I-bundle structure extends from A∪B to the the regular neighbourhood N(A∪B).If the bounding circles of A lie in distinct components of∂M,then the manifold M has to be homeomorphic to S1×S1×I.This is because each annulus V in the vertical boundary of the I-bundle N(A∪B)cuts offfrom M a3-ball of the form D×I where D is one of the discs in S−(∂A∪∂B).This3-ball can not contain A∪B since both annuli are incompressible.We can therefore extend the product structure over this3-ball,thus obtaining an I-bundle over the torus S.If both components of∂A live in S,then there are two possibilities for the compressible annulus V.The dichotomy comes from the discs D1and D2in the surface S,bounded by the circles of∂V. They can either be nested,say D1⊂int(D2),or disjoint.It is clear that the annulus A is disjoint from∂V=∂D1∪∂D2.The horizontal boundary of the regular neighbourhood N(A∪B)contains an embedded arc,running from∂D1to∂A,which is disjoint from∂D2.If thefirst of the two cases were true,this would imply that at least one of the boundary components of A is contained in D2,which is clearly a contradiction.So we must have an embedded2-sphere D1∪D2∪V which bounds a3-ball D1×I,disjoint from A∪B,like before.Adjoining all these solid cylinders to N(A∪B)makes our manifold M into an I-bundle with a single toral boundary component.But this has to be an I-bundle over a closed non-orientable surface of Euler characteristic zero,i.e.a Klein bottle.This concludes the proof.2A very useful consequence of theorem2.3that deals with orientable surfaces in M with zero Euler characteristic is contained in proposition2.5.Most of it is a direct consequence of corollary 6.8in[6].Proposition2.5Let M be an irreducible3-manifold with incompressible boundary that contains either an essential torus or an essential annulus.Let T be the triangulation of M.Then the following holds:(a)If A is a least weight normal representative(with respect to T)in an isotopy class of anessential torus in M,then every vertex surface in the face of the projective solution space that carries A is an essential torus.(b)Let A be a least weight essential annulus in M that is normal with respect to the triangula-tion T.Then there exists a vertex surface in M that is an essential annulus.If the boundary of M consists of tori only and if M is neither S1×S1×I nor an I-bundle over a Klein bottle,then the boundary of the vertex annulus is parallel to the boundary of A in∂M. Proof.Since vertex surfaces are two-sided by definition,(a)is just restating corollary6.8in[6].In (b)at least one of the vertex surfaces in the face of projective solution space has to be an annulus. In fact,by theorem2.3,it has to be an essential annulus.Assume now that M has toral boundary.All vertex annuli in the face of the projective solution space that carries A must have their respective boundaries lying in precisely the components of ∂M that contain∂A.Since all these vertex annuli are essential we can apply lemma2.4andfinish the proof.2 We will conclude this section by a short discussion of incompressible surfaces in Seifertfibred manifolds.A surface in a Seifertfibred space M→B is called vertical if it can be expressed as a union of regularfibres.So the only possibilities are a torus,a Klein bottle or an annulus.Moebius band does not come into the picture because the generator of its fundamental group can not be a regularfibre in M.On the other hand the surface that is transverse to allfibres in M is called horizontal.The following proposition says roughly that every essential surface in M→B has to be either vertical or horizontal.6Proposition2.6Let S be an incompressible∂-incompressible surface in a3-manifold M.Then the following holds:(a)Assume further that M→B is an irreducible Seifertfibred space,possibly containing nosingularfibres,over a(perhaps non-orientable)compact surface B.If S is two-sided and if it contains no singularfibres of M,then it is isotopic to either a vertical surface or a horizontal surface.(b)Let M→B be an S1-bundle over a(perhaps non-orientable)compact bounded surface B.If S is a one-sided,i.e.non-orientable,surface in M,then it is isotopic to either a vertical or a horizontal surface.It should be noted that(b)of proposition2.6actually fails if the base surface B is closed.It is not hard to see that the lens space L(2n,1),whichfibres as an S1-bundle over S2with Euler number2n,contains an incompressible surface homeomorphic to a connected sum of n projective planes.Such a surface can not be vertical because of its Euler characteristic,but it can also not be horizontal since the Euler number of our S1-bundle does not vanish.Also notice that the statement (b)for two-sided surfaces is already contained in(a).Proof.If the surface S contained a disc component,then,by the definition of incompressibility,∂M would have to compress.Since M is irreducible,this would make it into a solid torus.The surface S is then a disjoint collection of compression discs which is horizontal in anyfibration of the solid torus.So from now on we can assume that S contains no disc components.Part(a)of the proposition is a well-known fact about two-sided incompressible∂-incompressible surfaces(which contain no disc components)in Seifertfibred spaces.Its proof can be found in[5] (theorem VI.34).We shall now prove part(b).Let M→B be an S1-bundle over a compact bounded surface B.Choose disjoint arcs in B whose union decomposes B into a single disc.Let A be a union of disjoint vertical annuli in M that are the preimages of the collection of arcs under the bundle projection.Without loss of generality we can assume that the surface S was isotoped in M so that the number of components of S∩A is minimal.Then there are no simple closed curves in S∩A that are homotopically trivial in either S or A,because such curves can be used to reduce the number of components in S∩A(here we are using the fact that every bounded S1-bundle is irreducible). Similarly arcs in S∩A that are∂-parallel in either A or S do not occur because both surfaces are∂-incompressible and∂M is incompressible in M(otherwise M would be a solid torus and S would have to be a disc,which is not a one-sided surface).It follows now that S∩A consists only of vertical circles or horizontal arcs,i.e.spanning arcs in the annular components of A.Let M1be the solid torus M−int(N(A))and let S1be the surface M1∩S.There can be no trivial simple closed curves in(∂M)∩M1coming from∂S1,because S has no disc components.So we can conclude that∂S1consists either of horizontal or vertical circles in the torus∂M1(a horizontal simple closed curve is the one that intersects eachfibre in ∂M1transversely).The surface S1has to be incompressible in M1.Every compression disc D for S1in M1yields a disc D′in ing irreducibility of M we could isotope S(rel∂D)so that D′becomes D.If D′were not contained in S1,this move would reduce the number of pieces in S∩A.Claim.If∂S1consists of horizontal simple closed curves,then S1is a disjoint union of meridional discs in M1.It is enough to show that under the hypothesis of the claim the surface S1has to be∂-incompressible in M1.This is because the only connected incompressible∂-incompressible surface in a solid torus is its meridian disc.Assume to the contrary that S1is∂-compressible.Let D be a∂-compression disc for S1in M1.We will modify D,in a thin collar of∂M1,so that the arc D∩∂M1lies in an annulus from ∂M∩∂M1.This isotoped disc therefore lives in M and is a∂-compression disc for the surface S.Like before this leads to a contradiction,because we can use the isotoped disc to construct an isotopy in M that will reduce the number of components in S∩A.So to prove the claim we need to isotope the disc D.Letαbe the embedded arc D∩∂M1, running between two points from∂S1.Notice that∂M1is an alternating union of annuli coming7from two families:one is M 1∩∂M and the other one is M 1∩N (A ).We will now isotope αinto the interior of M 1∩∂M .Assume first that αis contained in the interior of an annulus from M 1∩N (A ).Then,since ∂S 1is horizontal in ∂M 1,the segments of ∂S 1in this annulus have to be spanning arcs.αcan either run between two distinct spanning arcs,or it can run around the annulus to hit the single spanning arc from two different sides.In either situation we can push αinto the interior of an adjacent annulus from M 1∩∂M .This isotopy can clearly be extended to the collar of ∂M 1,thus producing the desired ∂-compression disc.If,on the other hand,αis not contained in the interior of an annulus from ∂M 1,then we must somewhere have the situation as described by figure 2.of the boundary S M 1α1boundary ofannulus in disc EFigure 2:An annulus in ∂M 1containing the disc E with the following property:the intersection E ∩αis a subarc of αcontaining precisely one point from ∂α.We can construct an isotopy of α,and hence of D ,using the disc E ,that will reduce the number of points in the intersection α∩∂(M 1∩∂M ).By repeating this move we arrive at a contradiction and the claim follows.It follows directly from the claim that S 1with the horizontal boundary can be isotoped (rel ∂S 1)so that it is horizontal in the fibration of M 1.This isotopy induces an isotopy of S in M that makes it horizontal.If ∂S 1is vertical,S 1can only consist of annuli bounded by fibres of M 1.We can therefore isotope S 1(rel ∂S 1)so that it becomes vertical.This concludes the proof.23THE MAIN THEOREMLet’s start by defining precisely the class of Seifert fibred 3-manifolds that we shall consider.The manifold M has to be compact and orientable.It has to fibre into circles over a possibly non-orientable compact surface B .The case when M contains no singular fibres will also be considered.Since we are only interested in general Haken 3-manifolds,i.e.the ones that are irreducible and that contain an injective surface different from a 2-sphere,we need to exclude some Seifert fibred spaces.The ones to go first are S 1×S 2and the connected sum R P 3#R P 3since they are not irreducible (the latter manifold fibres as an S 1-bundle over a projective plane).Another obvious family that we have to disqualify are the lens spaces including the 3-sphere,because they contain no injective surfaces.Those are all Seifert fibred spaces over a 2-sphere with at most 2singular fibres.Among the spaces that contain no vertical essential tori are also manifolds that fibre over a projective plane with at most one singular fibre.Since the Klein bottle over the orientation reversing curve in R P 2is not injective,they contain no injective vertical surfaces.There are no horizontal surfaces either.The existence of such a surface would imply that the only singular fibre is of index 0。
Hermitian manifolds of pointwise constant antiholomorphic sectional curvatures

a rXiv:075.236v1[math.D G]2M a y27HERMITIAN MANIFOLDS OF POINTWISE CONSTANT ANTIHOLOMORPHIC SECTIONAL CURVATURES GEORGI GANCHEV AND OGNIAN KASSABOV Abstract.In dimension greater than four,we prove that if a Hermitian non-Kaehler manifold is of pointwise constant antiholomorphic sectional curvatures,then it is of constant sectional curvatures. 1.Introduction Let (M,g,J )(dim M =2n ≥4)be an almost Hermitian manifold.Any two-plane (sec-tion)E in the tangential space T p M,p ∈M determines an angle θ=∠(E,JE ),θ∈[0,π2or E ⊥JE .The latter are also known as totally real in view of the condition E ⊥JE .If Φis the fundamental K¨a hler form of the manifold,then any antiholomrphic section E is characterized by the condition Φ|E =0.Because of this characterization,these tangent planes are also known as Lagrangean .An almost Hermitian manifold is said to be of pointwise constant antiholomorphic sec-tional curvature νif the Riemannian sectional curvature K (E ;p )does not depend on the antiholomorphic section E in T p M,p ∈M ,i.e.K (E ;p )=ν(p )is only a function of the point p ∈M .A tensor characterization for an almost Hermitian manifold of pointwise constant anti-holomorphic sectional curvature in dim M ≥4has been found in [2].In [4]it has been proved that the antiholomorphic sectional curvature ν(p )is a constant on the manifold under the condition dim M >4.A complete classification of compact Hermitian surfaces (dim M =4)with pointwise constant antiholomorphic sectional curvature has been given in [1].Four-dimensional almost Hermitian manifolds of pointwise constant antiholomorphic sectional curvature have been studied in [5].In this paper we consider the class of Hermitian manifolds and prove our mainTheorem A .If a Hermitian non-K¨a hler manifold with a real dimension greater than four is of pointwise constant antiholomorphic sectional curvature,then the manifold is of constant sectional curvature.2.PreliminariesLet (M,g,J )(dim M =2n ≥4be an almost Hermitian manifold with metric g and almost complex structure J .The tangent space to M at an arbitrary point p ∈M is denoted by2GEORGI GANCHEV AND OGNIAN KASSABOVT p M and the algebra of all differentiable vector fields on M is denoted by X M .The K¨a hler form Φof the structure (g,J )is defined by the equalityΦ(X,Y )=g (JX,Y ),X,Y ∈T p M,p ∈M.The Levi-Civita connection of the metric g is denoted by ∇and the Riemannian curvature tensor R of type (1,3)is given by R (X,Y )Z =∇X ∇Y Z −∇Y ∇X Z −∇[X,Y ]Z,X,Y,Z ∈X M.The corresponding curvature tensor of type (0,4)is given by R (X,Y,Z,U )=g (R (X,Y )Z,U ),for all vector fields X,Y,Z,U .Let {e 1,...,e 2n }be an orthonormal basis at a point p ∈M .The Ricci tensor ρand the scalar curvature τof the metric g are determined as followsρ(X,Y )=2n i =1R (e i ,X,Y,e i ),τ=2n i =1ρ(e i ,e i );X,Y ∈T p M.The almost complex structure (g,J )gives rise to the ∗-Ricci tensor ρ∗and to the ∗-scalar curvature τ∗defined by the formulasρ∗(X,Y )=2n i =1R (e i ,X,JY,Je i ),τ∗=2n i =1ρ∗(e i ,e i );X,Y ∈T p M.While the Ricci tensor is symmetric,the ∗-Ricci tensor has the property(2.1)ρ∗(JX,JY )=ρ(Y,X ),X,Y ∈T p M.The following tensor of type (0,3)F (X,Y,Z )=g ((∇X J )Y,Z ),X,Y,Z ∈X Mis closely related to the structure (g,J ).This tensor satisfies the following properties (2.2)F (X,Y,Z )=−F (X,Z,Y ),F (X,JY,JZ )=−F (X,Y,Z ).The well known classes of almost Hermitian manifolds have been obtained in terms of the properties of the tensor F in [3].In this section we consider Hermitian manifolds,which are characterized by the following property of the tensor F [3]:(2.3)(∇JX J )Y =J (∇X J )Y ⇐⇒F (JX,Y,Z )=−F (X,JY,Z ).Let T C p M be the complexification of the tangent space T p M at any point p ∈M .By X C M we denote the algebra of complex differentiable vector fields on M .The complex structure J generates the standard splittingsT C p M =T 1,0p M ⊕T 0,1p M,X C M =X 1,0M ⊕X 0,1M.If {e 1,...,e n ;Je 1,...,Je n }is an orthonormal frame at a point p ∈M ,then the vectors Z α=e α−iJe α2;α=1,...,n form a basis for T 1,0p M and T 0,1p M ,respectively.Further,we call these bases {Z α;Z ¯α}α=1,...,n special complex bases .For an arbitrary tensor T we denote T α...=T (Z α...)and T ¯α...=T (Z ¯α...).In what follows,the summation convention is assumed and Greek indices α,β,γ,...run from 1to n .HERMITIAN MANIFOLDS OF CONSTANT ANTIHOLOMORPHIC SECTIONAL CURVATURES 3It follows that the components of the metric tensor with respect to a special complex basis satisfy the conditionsg αβ=0(for all α,β);g α¯β=0,(for α=β);g α¯α=12(n +1)Ψ(ρ∗)+τ∗2n +1π2 .We introduce the tensor Q =14(n +1)(2n +1)g ,which in view of (2.1)has the property (2.4).Then the condition (2.5)can be written as follows:(2.6)R =Ψ(Q )+νπ1.The second author has proved in[4]that in dim M ≥6the function ν(p )in (2.5)is con-stant.Thus,we shall speak about almost Hermitian manifolds of constant antiholomorphic sectional curvature instead of ”pointwise constant”antiholomorphic sectional curvature.4GEORGI GANCHEV AND OGNIAN KASSABOV3.Proof of Theorem AIn this section we prove Theorem A on the base of the following statement.Proposition3.1.Let(M,g,J)(dim C M≥3)be a Hermitian manifold of constant anti-holomorphic sectional curvature.Then any non-K¨a hler point of M has a neighborhood in which(M,g,J)is of constant sectional curvature.Proof:Let p0be a point in M with F=0at p0.We consider a neighborhood U of p0, such that F=0at any point of U.We shall prove that(M,g,J)is of constant sectional curvature in U.For any p∈U,we consider a special complex basis{Zα,Z¯α}α=1,...,n at the point p. The property(2.4)of the tensor Q implies that(3.1)Qα¯β=Q¯βα,Qαβ=−Qβα.Taking into account the property(2.3)of the covariant derivative of the complex structure and the symmetry(2.4)of the tensor Q,we compute(3.2)(∇X Q)(JY,JZ)=(∇X Q)(Z,Y)−Q((∇X J)Y,JZ)−Q(JY,(∇X J)Z)for arbitrary X,Y,Z∈X U.Since the tensor F has the symmetries(2.2)and(2.3),then its essential components(those which may not be zero)with respect to a special complex basis{Zα,Z¯α}are only F¯αβγand their conjugates.These components satisfy the condition F¯αβγ=−F¯αγβ.These properties of the tensor F can be expressed in terms of the covariant derivative(∇X J)Y as follows(3.3)∇αJγβ=∇¯αJγβ=∇αJ¯γβ=0.The equalities(3.2)and(3.3)imply that(3.4)∇αQ¯γβ=∇αQβ¯γ+i∇αJσ¯γQβσ;(3.5)∇αQβγ=−∇αQγβ,(especially∇αQββ=0);(3.6)∇¯αQββ=i∇¯αJ¯σβQ¯σβ.First we prove the following statementLemma3.2.Let Z,W∈T1,0pM and g(Z,¯W)=0.If F(¯Z,Z,W)=0,then Q(Z,W)=0. Proof:Since g(Z,¯W)=0,then we canfind a special complex basis{Zα,Z¯α}α=1,...,n such that the vectors Z and W are collinear with Zαand Zβ,respectively,for someα=β. Applying the Bianchi identity for the curvature tensor R in the form∇αRβγβ¯γ+∇βRγαβ¯γ+∇γRαββ¯γ=0,wefind(3.7)∇βQαβ=0.Further we apply the Bianchi identity in the form∇¯αRαβαβ+∇αRβ¯ααβ+∇βR¯αααβ=0and taking into account(3.7),we obtain(3.8)F¯ααβQαβ=0.HERMITIAN MANIFOLDS OF CONSTANT ANTIHOLOMORPHIC SECTIONAL CURVATURES5 Under the conditions of the lemma we have F¯ααβ=0.Then it follows from(3.8)that Qαβ=0. Next we proveLemma3.3.The tensor Q is symmetric at any point p∈U.Proof:Since the tensor F=0at the point p,then because of the Lemma2.1there exist indicesα=βso that F¯ααβ=0.Applying Lemma3.2,it follows that Qαβ=0.Letγ=α,β.Since F¯ααβ=0,then the complex function w(t)=F(Z¯α,Zα,Zβ+tZγ)=0 for all sufficiently small t∈R.It follows from Lemma3.2that Q(Zα,Zβ+tZγ)=0.Hence, Q(Zα,Zγ)=0,i.e.Qαγ=0.Similarly,the inequality F(Zα+tZγ,Zα+tZγ,Zβ)=0for all sufficiently small real t and Lemma3.2imply that Q(Zα+tZγ,Zβ)=0.Hence,Qγβ=0.So far,we obtainedQαβ=Qαγ=Qβγ=0.In dim M>6,letδ=α,β,γ.As in the above,wefindQαδ=Qαδ=Qβδ=0.On the other hand,the inequality F(Z¯α+tZ¯γ,Zα+tZγ,Zβ+tZδ=0),which is valid for sufficiently small real t,implies that Qγδ=0.Thus we obtained Qλµ=0for allλ,µ=1,...,n,which proves the assertion. Finally,we shall prove that the tensor Q is proportional to the metric tensor g in U. For that purpose it is sufficient to prove that(3.9)Qλ¯µ=0,for all different indicesλandµ.We consider two cases for the tensor F=0:1)There exist three different indicesα,β,γ,such that F¯γαβ=0;2)F¯γαβ=0for all different indicesα,β,γwith respect to any special complex basis. The case1).Applying the second Bianchi identity in the form∇αRβ¯γβ¯γ+∇βR¯γαβ¯γ+∇¯γRαββ¯γ=0,we get the equality F¯γαβQβ¯γ=0,which implies that Qβ¯γ=0.Now,arguments similar to those in Lemma3.3show(3.9).The case2).According to Lemma2.1there exist two different indicesαandβsuch that F¯ααβ=0.Applying the second Bianchi identity in the form∇αR¯γββ¯α+∇¯γRβαβ¯α+∇βRα¯γβ¯α=0,and taking into account the equalities Qαβ=0,F¯γαβ=0,wefind−∇¯γQββ+iQ(Zβ,(∇¯γJ)Zβ)+∇βQ¯γβ=0.The last equality in view of(3.6)implies(3.10)∇βQ¯γβ=0.Applying the second Bianchi identity in the form∇¯αRαββ¯γ+∇αRβ¯αβ¯γ+∇βR¯ααβ¯γ=0,6GEORGI GANCHEV AND OGNIAN KASSABOVwefind3iF¯ααβQβ¯γ+2∇βQβ¯γ+2iQ(Zβ,(∇βJ)Z¯γ)=0,which together with(3.4)gives3iF¯ααβQβ¯γ+2∇βQ¯γβ=0.The last equality and(3.10)imply thatF¯ααβQβ¯γ=0.Hence,Qβ¯γ=0.Applying again the scheme of the proof of Lemma3.3,we obtain the conditions(3.9).Thus,in both cases1)and2),we obtained the conditions(3.9),which are equivalent to the identity(3.11)Q(X,Y)=0,whenever X,Y∈T p M,g(X,Y)=0.Applying standard arguments for the symmetric tensor Q(X,Y),we obtain that the tensor Q is proportional to the metric tensor g,i.e.Q=tr Q2(2n+1).HenceR=νπ1+tr Q2n(2n−1)=τ∗HERMITIAN MANIFOLDS OF CONSTANT ANTIHOLOMORPHIC SECTIONAL CURVATURES7References[1]Apostolov V.,Ganchev G.,Ivanov pact Hermitian Surfaces of Constant Antiholomorphic SectionalCurvatures.Proc.Amer.Math.Soc.,125(1997)12,3705-3714.[2]Ganchev G.On Bochner curvature tensors in almost Hermitian manifolds.Pliska,Studia mathematicabulgarica,9(1987),33-42.[3]Gray A.,Hervella L.The sixteen classes of almost Hermitian manifolds and their linear invariants.Ann.di Mat.Pura ed Appl.,123(1980),35-58.[4]Kassabov O.Schur’s Theorem for almost Hermitian manifolds.C.R.Acad.bulg.Sci.,54(2001),15-18.[5]Sato T.Almost Hermitian4-manifolds of pointwise constant antiholomorphic sectional curvature.J.Geom.,77(2003)1-2,171-183.[6]Tricerri F.,Vanhecke,L.Curvature tensors on almost Hermitian manifolds,Trans.Amer.Math.Soc.,267(1981),365-398.Bulgarian Academy of Sciences,Institute of Mathematics and Informatics,Acad.G. Bonchev Str.bl.8,1113Sofia,BulgariaE-mail address:ganchev@math.bas.bgHigher transport School,Sofia,BulgariaE-mail address:okassabov@vtu.bg。
佩雷尔曼关于庞加莱猜想的论文0307245

a rXiv:mat h .DG /37245v117J ul23Finite extinction time for the solutions to the Ricci flow on certain three-manifolds Grisha Perelman ∗May 21,2006In our previous paper we constructed complete solutions to the Ricci flow with surgery for arbitrary initial riemannian metric on a (closed,oriented)three-manifold [P,6.1],and used the behavior of such solutions to classify three-manifolds into three types [P,8.2].In particular,the first type consisted of those manifolds,whose prime factors are diffeomorphic copies of spherical space forms and S 2×S 1;they were characterized by the property that they admit metrics,that give rise to solutions to the Ricci flow with surgery,which become extinct in finite time.While this classification was sufficient to answer topological ques-tions,an analytical question of significant independent interest remained open,namely,whether the solution becomes extinct in finite time for every initial metric on a manifold of this type.In this note we prove that this is indeed the case.Our argument (in con-junction with [P,§1-5])also gives a direct proof of the so called ”elliptization conjecture”.It turns out that it does not require any substantially new ideas:we use only a version of the least area disk argument from [H,§11]and a regu-larization of the curve shortening flow from [A-G].1Finite time extinction 1.1Theorem.Let M be a closed oriented three-manifold,whose prime decom-position contains no aspherical factors.Then for any initial metric on M thesolution to the Ricci flow with surgery becomes extinct in finite time.Proof for irreducible M .Let ΛM denote the space of all contractible loops in C 1(S 1→M ).Given a riemannian metric g on M and c ∈ΛM,define A (c,g )to be the infimum of the areas of all lipschitz maps from D 2to M,whose restriction to ∂D 2=S 1is c.For a family Γ⊂ΛM let A (Γ,g )be the supremum of A (c,g )over all c ∈Γ.Finally,for a nontrivial homotopy class α∈π∗(ΛM,M )let A (α,g )be the infimum of A (Γ,g )over all Γ∈α.Since M is not aspherical,it follows from a classical (and elementary)result of Serre that such a nontrivial homotopy class exists.1.2Lemma.(cf.[H,§11])If g t is a smooth solution to the Ricciflow,then for anyαthe rate of change of the function A t=A(α,g t)satisfies the estimatedR t min A t2(in the sense of the lim sup of the forward difference quotients),where R t min denotes the minimum of the scalar curvature of the metric g t.A rigorous proof of this lemma will be given in§3,but the idea is simple and can be explained here.Let us assume that at time t the value A t is attained by the familyΓ,such that the loops c∈Γwhere A(c,g t)is close to A t are embedded and sufficiently smooth.For each such c consider the minimal disk D c with boundary c and with area A(c,g t).Now let the metric evolve by the Ricciflow and let the curves c evolve by the curve shorteningflow(which moves every point of the curve in the direction of its curvature vector at this point) with the same time parameter.Then the rate of change of the area of D c can be computed as(−Tr(Ric T))+ c(−k g)D cwhere Ric T is the Ricci tensor of M restricted to the tangent plane of D c,and k g is the geodesic curvature of c with respect to D c(cf.[A-G,Lemma3.2]).In three dimensions thefirst integrand equals−1R−K)+ c(−k g)= D c(−12the conclusion of the lemma above holds for the solutions to the Ricciflow with surgery as well.Now recall that the evolution equation for the scalar curvatured3R2+2|Ric◦|2implies the estimate R t min≥−3t+const.It follows thatˆA t=A tdtˆA t≤−2πdt c t(x)=H t(x),where x is the parameter on S1,and H t is the curvaturevectorfield of c t with respect to g t.It is known[G-H]that for any smoothly immersed initial curve c the solution c t exists on some time interval[t0,t′1),each c t for t>t0is an analytic immersed curve,and either t′1=t1,or the curvature k t=g t(H t,H t)1Denote by X t the tangent vectorfield to c t,and let S t=g t(X t,X t)−1g(X,X)=−2Ric(X,X)−2g(X,X)k2,(1)dtwhich implies[H,S]=(k2+Ric(S,S))S(2) Now we can computedk≤k′′+k3+const·(k+1)(4)dtNow it follows from(1)and(4)that the length L and the total curvature Θ= kds satisfy dΘ≤ const·(k+1)ds(6)dtIn particular,both quantities can grow at most exponentially in t(they would be non-increasing in aflat manifold).2.2In general the curvature of c t may concentrate near certain points,cre-ating singularities.However,if we know that this does not happen at some time t∗,then we can estimate the curvature and higher derivatives at times shortly thereafter.More precisely,there exist constantsǫ,C1,C2,...(which may depend on the curvatures of the ambient space and their derivatives,but are independent of c t),such that if at time t∗for some r>0the length of c t is at least r and the total curvature of each arc of length r does not exceedǫ,then for every t∈(t∗,t∗+ǫr2)the curvature k and higher derivatives satisfy the estimates k2=g(H,H)≤C0(t−t∗)−1,g(∇S H,∇S H)≤C1(t−t∗)−2,... This can be proved by adapting the arguments of Ecker and Huisken[E-Hu]; see also[A-G,§4].2.3Now suppose that our manifold(M,g t)is a metric product(¯M,¯g t)×S1λ, where the second factor is the circle of constant lengthλ;let U denote the unit tangent vectorfield to this factor.Then u=g(S,U)satisfies the evolution equationdAssume that u was strictly positive everywhere at time t0(in this case the curve is called a ramp).Then it will remain positive and bounded away from zero as long as the solution exists.Now combining(4)and(7)we can estimate the right hand side of the evolution equation for the ratio kµt≤(2n−1)|Rm t|µt,(8)dtwhere|Rm t|denotes a bound on the absolute value of sectional curvatures of g t.Indeed,the curves c t1and c t2,being ramps,are embedded and without substantial loss of generality we may assume them to be disjoint.In this case the results of Morrey[M]and Hildebrandt[Hi]yield an analytic minimal annulus A, immersed,except at mostfinitely many branch points,with prescribed boundary and with areaµ.The rate of change of the area of A can be computed as(−Tr(Ric T))+ ∂A(−k g)≤ A(−Tr(Ric T)+K)A≤ A(−Tr(Ric T)+Rm T)≤(2n−1)|Rm|µ,where thefirst inequality comes from the Gauss-Bonnet theorem,with possible contribution of the branch points,and the second one is due to the fact that a minimal surface has nonpositive extrinsic curvature with respect to any normal vector.2.5The estimate(8)implies thatµt can grow at most exponentially;in particular,if c t1and c t2were very close at time t0,then they would be close for all t∈[t0,t1]in the sense of minimal annulus area.In general this does not imply that the lengths of the curves are also close.However,an elementary argument shows that ifǫ>0is small then,given any r>0,one canfind¯µ, depending only on r and on upper bound for sectional curvatures of the ambient space,such that if the length of c t1is at least r,each arc of c t1with length r has total curvature at mostǫ,andµt≤¯µ,then L(c t2)≥(1−100ǫ)L(c t1).3Proof of lemma1.23.1In this section we prove the following statementLet M be a closed three-manifold,and let(M,g t)be a smooth solution to the Ricciflow on afinite time interval[t0,t1].Suppose thatΓ⊂ΛM is a compact family.Then for anyξ>0one can construct a continuous deformationΓt,t∈[t0,t1],Γt0=Γ,such that for each curve c∈Γeither the value A(c t1,g t1)is bounded from above byξplus the value at t=t1of the solution to the ODE5d2R t min w(t)with the initial data w(t0)=A(c t0,g t0),orL(c t1)≤ξ;moreover,if c was a constant map,then all c t are constant maps.It is clear that our statement implies lemma1.2,because a family consisting of very short loops can not represent a nontrivial relative homotopy class.3.2As afirst step of the proof of the statement we can replaceΓby a family,which consists of piecewise geodesic loops with some largefixed number of vertices and with each segment reparametrized in some standard way to make the parametrizations of the whole curves twice continuously differentiable.Now consider the manifold Mλ=M×S1λ,0<λ<1,and for each c∈Γconsider the smooth embedded closed curve cλsuch that p1cλ(x)=c(x)and p2cλ(x)=λx modλ,where p1and p2are projections of Mλto thefirst and second factor respectively,and x is the parameter of the curve c on the standard circle of length ing2.3we can construct a solution c tλ,t∈[t0,t1]to the curve shorteningflow with initial data cλ.The required deformation will be obtained asΓt=p1Γtλ(whereΓtλdenotes the family consisting of c tλ)for certain sufficiently smallλ>0.We’ll verify that an appropriateλcan be found for each individual curve c,or for anyfinite number of them,and then show that if ourλworks for all elements of aµ-net inΓ,for sufficiently smallµ>0, then it works for all elements ofΓ.3.3In the following estimates we shall denote by C large constants that may depend on metrics g t,familyΓandξ,but are independent ofλ,µand a particular curve c.Thefirst step in3.2implies that the lengths and total curvatures of cλare uniformly bounded,so by2.1the same is true for all c tλ.It follows that the area swept by c tλ,t∈[t′,t′′]⊂[t0,t1]is bounded above by C(t′′−t′),and therefore we have the estimates A(p1c tλ,g t)≤C,A(p1c t′′λ,g t′′)−A(p1c t′λ,g t′)≤C(t′′−t′).3.4It follows from(5)that t1t0 k2dsdt≤C for any c tλ.Fix some large constant B,to be chosen later.Then there is a subset I B(cλ)⊂[t0,t1]of measure at least t1−t0−CB−1where k2ds≤B,hence kds≤ǫon any arc of length≤ǫ2B−1.Assuming that c tλare at least that long,we can apply2.2 and construct another subset J B(cλ)⊂[t0,t1]of measure at least t1−t0−CB−1, consisting offinitely many intervals of measure at least C−1B−2each,such that for any t∈J B(cλ)we have pointwise estimates on c tλfor curvature and higher derivatives,of the form k≤CB,...Nowfix c,B,and consider any sequence ofλ→0.Assume again that the lengths of c tλare bounded below byǫ2B−1,at least for t∈[t0,t2],where t2= t1−B−1.Then an elementary argument shows that we canfind a subsequence Λc and a subset J B(c)⊂[t0,t2]of measure at least t1−t0−CB−1,consisting offinitely many intervals,such that J B(c)⊂J B(cλ)for allλ∈Λc.It follows that on every interval of J B(c)the curve shorteningflows c tλsmoothly converge (asλ→0in some subsequence ofΛc)to a curve shorteningflow in M.Let w c(t)be the solution of the ODE d2R t min w c(t)withinitial data w c(t0)=A(c,g t0).Then for sufficiently smallλ∈Λc we have A(p1c tλ,g t)≤w c(t)+1disk argument as in 1.2,and this implies the corresponding estimate for p 1c t λif λ∈Λc is small enough,whereas for the intervals of the complement of J B (c )we can use the estimate in 3.3.On the other hand,if our assumption on the lower bound for lengths does not hold,then it follows from (5)that L (c t 2λ)≤CB−1≤12ξor L (ˆc t 2λ)≤12ξand µ≤C −1ξ,then A (p 1c t1λ,g t 1)≤w c (t 1)+ξ.Onthe other hand,if L (ˆc t2λ)≤14ξfor all t ∈[t 2,t 1];on the other hand,using (5)we can find a t ∈[t 2,t 1],such that k 2ds ≤CB for c t λ;hence,applying 2.5,we get L (ˆc t λ)>22ξ.The proof of the statement3.1is complete.References[A-G]S.Altschuler,M.Grayson Shortening space curves and flow through singularities.Jour.Diff.Geom.35(1992),283-298.[B]S.Bando Real analyticity of solutions of Hamilton’s equation.Math.Zeit.195(1987),93-97.[E-Hu]K.Ecker,G.Huisken Interior estimates for hypersurfaces moving by mean curvature.Invent.Math.105(1991),547-569.[G-H]M.Gage,R.S.Hamilton The heat equation shrinking convex plane curves.Jour.Diff.Geom.23(1986),69-96.[H]R.S.Hamilton Non-singular solutions of the Ricci flow on mun.Anal.Geom.7(1999),695-729.[Hi]S.Hildebrandt Boundary behavior of minimal surfaces.Arch.Rat.Mech.Anal.35(1969),47-82.[M]C.B.Morrey The problem of Plateau on a riemannian manifold.Ann.Math.49(1948),807-851.[P]G.Perelman Ricci flow with surgery on three-manifolds.arXiv:math.DG/0303109v17。
四象限法则英文

四象限法则英文The Four Quadrant Rule。
The Four Quadrant Rule is a powerful concept that can help individuals and organizations make better decisions and manage their time more effectively. This rule is based on the idea that all activities and tasks can be categorized into four quadrants based on their urgency and importance. By understanding and applying this rule, individuals can prioritize their tasks and focus on the activities that will have the greatest impact on their goals and objectives.The Four Quadrant Rule was popularized by Stephen Covey in his book "The 7 Habits of Highly Effective People." Covey explained that all activities can be classified into one of four quadrants based on two factors: urgency and importance. The four quadrants are:1. Quadrant 1: Urgent and Important These are the tasksthat require immediate attention and have a significant impact on your goals and objectives. Examples include deadlines, crises, and emergencies.2. Quadrant 2: Not Urgent but Important These are the tasks that are important for long-term success but do not require immediate attention. Examples include planning, relationship building, and personal development.3. Quadrant 3: Urgent but Not Important These are the tasks that demand immediate attention but do not contribute to long-term success. Examples include interruptions, unnecessary meetings, and some emails.4. Quadrant 4: Not Urgent and Not Important These are the tasks that are neither urgent nor important. Examples include time-wasting activities, trivial tasks, and distractions.By categorizing tasks and activities into these four quadrants, individuals can gain a clearer understanding of where they should focus their time and energy. The goal isto spend more time in Quadrant 2, where important but not urgent tasks can be addressed proactively and prevent them from becoming urgent and important. This allows individuals to be more strategic and proactive in their approach to managing their time and priorities.The Four Quadrant Rule can be applied to both personal and professional life. In a personal context, individuals can use this rule to prioritize their daily tasks and focus on activities that align with their long-term goals and values. For example, spending time with family, exercising, and pursuing personal growth opportunities would fall into Quadrant 2. By prioritizing these activities, individuals can lead a more balanced and fulfilling life.In a professional context, the Four Quadrant Rule can help individuals and teams prioritize their work and make better decisions about how to allocate their time and resources. By focusing on Quadrant 1 and Quadrant 2 activities, organizations can improve their productivity, reduce stress, and achieve better results. This rule can also be used to identify and eliminate time-wastingactivities and distractions that do not contribute to the organization's success.One of the key benefits of the Four Quadrant Rule is that it helps individuals and organizations avoid the trap of being constantly reactive and firefighting. By spending more time in Quadrant 2, individuals can be more proactive and prevent urgent and important tasks from dominating their time and attention. This can lead to better decision-making, improved productivity, and a greater sense of control over one's life and work.In order to effectively apply the Four Quadrant Rule, individuals should regularly assess their tasks and activities and categorize them into the appropriate quadrants. This can be done through a simple exercise of listing out all the tasks and activities and evaluating their urgency and importance. By doing this, individuals can gain clarity on where they should focus their time and energy.It's important to note that the Four Quadrant Rule isnot a one-size-fits-all solution. Different individuals and organizations may have different priorities and values, and what may be important for one person may not be as important for another. Therefore, it's important for individuals to adapt this rule to their own unique circumstances and make adjustments as needed.In conclusion, the Four Quadrant Rule is a valuabletool for individuals and organizations to prioritize their tasks and manage their time more effectively. By categorizing activities into four quadrants based on urgency and importance, individuals can gain clarity on where to focus their time and energy. This can lead to improved productivity, better decision-making, and a greater sense of control over one's life and work. Whether applied in a personal or professional context, the Four Quadrant Rule can help individuals achieve their goals and lead a more balanced and fulfilling life.。
differential manifold 英文书籍

differential manifold 英文书籍A Brief Introduction to Differential ManifoldsIntroductionDifferential manifold theory is an essential branch of mathematics and plays a crucial role in various disciplines such as physics and engineering. In this article, we will explore the concept of a differential manifold, its properties, and its applications. This article aims to provide a concise yet comprehensive understanding of differential manifolds for readers interested in the subject.What is a Differential Manifold?A differential manifold is a mathematical space that locally resembles Euclidean space but may have a complicated global structure. It can be defined in terms of charts, which are coordinate systems that map points in the manifold to points in Euclidean space. These charts allow us to define smooth functions on the manifold, and the compatibility conditions between different charts ensure a smooth transition between coordinate systems.Properties of Differential ManifoldsOne of the fundamental properties of differential manifolds is their dimension. A manifold can be n-dimensional if it can be locally parametrized by n real numbers. The local linearity property of manifolds ensures that tangent vectors can be defined at each point, allowing for the study of derivatives and vector fields.Another key property of differential manifolds is their topology. A manifold can be either open or closed, connected or disconnected, compact or non-compact, depending on its underlying topology. The notion of continuity and differentiability can be extended to functions defined on manifolds, leading to the study of smooth maps and flows.Applications of Differential ManifoldsDifferential manifold theory finds applications in numerous fields, including physics, engineering, and computer science. In physics, differential manifolds provide a geometric framework for studying space-time in general relativity. The curvature and topology of manifolds play a crucial role in understanding the gravitational field.In engineering, differential manifolds are used to model and analyze various systems. Electrical circuits, control systems, and signal processing can all be represented as manifolds, allowing for the application of differential equations and control theory.In computer science, differential manifolds are employed in computer vision, robotics, and machine learning. Manifold learning algorithms aim to uncover the underlying structure and dimensionality of high-dimensional data by mapping it onto a lower-dimensional manifold.ConclusionIn conclusion, differential manifolds are fascinating mathematical objects that provide a flexible framework for studying smooth functions, derivatives, and geometric properties. They have broad applications in various scientific and engineering fields, making them an indispensable toolfor understanding and solving complex problems. By grasping the fundamental concepts and properties of differential manifolds, readers can delve into more advanced topics and further explore the beauty and elegance of this mathematical discipline.。
西尔维斯特的四点问题
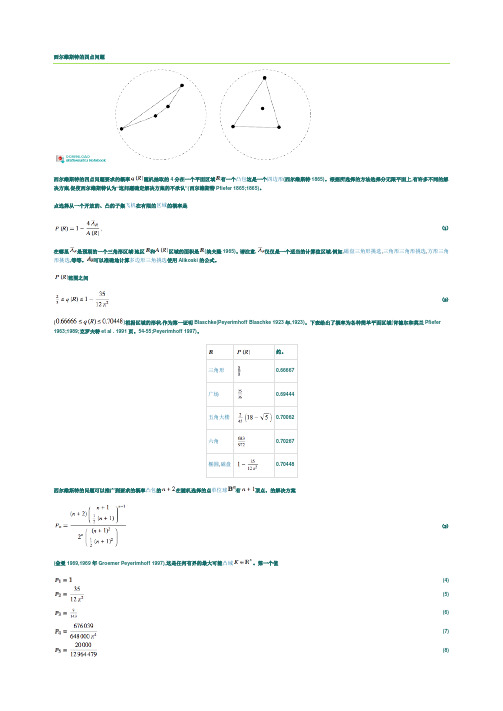
西尔维斯特的四点问题西尔维斯特的四点问题要求的概率随机抽取的4分在一个平面区域有一个凸包这是一个四边形(西尔维斯特1865)。
根据所选择的方法选择分无限平面上,有许多不同的解决方案,促使西尔维斯特认为“这问题确定解决方案的不承认”(西尔维斯特Pfiefer 1865;1865)。
点选择从一个开放的、凸的子集飞机在有限的区域的概率是(1)在哪里是预期的一个三角形区域/地区和区域的面积是(埃夫隆1965)。
请注意,仅仅是一个适当的计算值区域,例如,磁盘三角形挑选,三角形三角形挑选,方形三角形挑选,等等。
可以准确地计算多边形三角挑选使用Alikoski的公式。
范围之间(2)()根据区域的形状,作为第一证明Blaschke(Peyerimhoff Blaschke 1923年,1923)。
下表给出了概率为各种简单平面区域(肯德尔和莫兰Pfiefer 1963;1989;克罗夫特et al . 1991页。
54-55;Peyerimhoff 1997)。
约。
0.666670.694440.700620.70267磁盘0.70448西尔维斯特的问题可以推广到要求的概率凸包的在随机选择的点单位球有顶点。
的解决方案(3) (金曼1969,1969年Groemer Peyerimhoff 1997),这是任何有界的最大可能凸域。
第一个值(4)(5)(6)(7)(8)(OEIS A051050和A051051).另一个泛化的概率问道随机选择的点在一个固定的有界凸域是一个凸的顶点百分度。
解决方案是(9)三角域,也有前几值1,1,1,2/3,11/36,91/900,17/675,…(OEIS A004677和A004824),(10)一个平行四边形域,头几个值1,1,1,25/36,49/144,121/3600,…(OEIS A004936和A005017;1996年Valtr Peyerimhoff 1996)。
史蒂芬 威廉森 宏观经济学 第四版 课后题答案 最新Solution_CH4

Chapter 4Consumer and Firm Behavior: The Work-Leisure Decision and Profit MaximizationTextbook Question SolutionsQuestions for Review1. Consumers consume an aggregate consumption good and leisure.2. Consumers’ preferences are summarized in a utility function.3. The first property is that more is always preferred to less. This property assures us that a consumptionbundle with more of one good and no less of the other good than any second bundle will always be preferred to the second bundle.The second property is that a consumer likes diversity in his or her consumption bundle. Thisproperty assures us that a linear combination of two consumption bundles will always be preferred to the two original bundles.The third property is that both consumption and leisure are normal goods. This property assures us that an increase in a consumer’s income will always induce the individual to consume more of both consumption and leisure.4. The first property of indifference curves is that they are downward sloping. This property is a directconsequence of the property that more is always preferred to less. The second property ofindifference curves is that they are bowed toward the origin. This property is a direct consequence of consumers’ preference for diversity.5. Consumers maximize the amount of utility they can derive from their given amount of availableresources.6. The optimal bundle has the property that it represents a point of tangency of the budget line with anindifference curve. An equivalent property is that the marginal rate of substitution of leisure forconsumption and leisure is equal to the real wage.7. In response to an increase in dividend income, the consumer will consume more goods and moreleisure.8. In response to an increase in the real value of a lump-sum tax, the consumer will consume less goodsand less leisure.28 Williamson • Macroeconomics, Fourth Edition9. An increase in the real wage makes the consumer more well off. As a result of this pure income effect,the consumer wants more leisure. Alternatively, the increase in the real wage induces a substitution effect in which the consumer is willing to consume less leisure in exchange for working more hours (consuming less leisure). The net effect of these two competing forces is theoretically ambiguous.10. The representative firm seeks to maximize profits.11. As the amount of labor is increased, holding the amount of capital constant, each worker gets asmaller share of the fixed amount of capital, and there is a reduction in each worker’s marginalproductivity.12. An increase in total factor productivity shifts the production function upward.13. The representative firm’s profit is equal to its production (revenue measured in units of goods) minusits variable labor costs (the real wage times the amount of labor input). A unit increase in labor input adds the marginal product of labor to revenue and adds the real wage to labor costs. The amount of labor demand is that amount of labor input that equates marginal revenue with marginal labor costs.This quantity of labor, labor demand, can simply be read off the marginal product of labor schedule.Problems1. Consider the two hypothetical indifference curves in the figure below. Point A is on both indifferencecurves, I1 and I2. By construction, the consumer is indifferent between A and B, as both points are on I2. In like fashion, the consumer is indifferent between A and C, as both points are on I1. But atpoint C, the consumer has more consumption and more leisure than at point B. As long as theconsumer prefers more to less, he or she must strictly prefer C to A. We therefore contradict thehypothesis that two indifference curves can cross.2. u al bC=+(a) To specify an indifference curve, we hold utility constant at u Next rearrange in the form:u aC l=−b bChapter 4 Consumer and Firm Behavior: The Work-Leisure Decision and Profit Maximization 29 Indifference curves are therefore linear with slope, −a /b , which represents the marginal rate ofsubstitution. There are two main cases, according to whether /a b w > or /.a b w < The top panelof the left figure below shows the case of /.a b w < In this case the indifference curves are flatterthan the budget line and the consumer picks point A, at which 0l = and .C wh T π=+− Theright figure shows the case of /.a b w > In this case the indifference curves are steeper than thebudget line, and the consumer picks point B, at which l h = and .C T π=− In the coincidentalcase in which /,a b w = the highest attainable indifference curve coincides with the indifference curve, and the consumer is indifferent among all possible amounts of leisure and hours worked.(b) The utility function in this problem does not obey the property that the consumer prefers diversity,and is therefore not a likely possibility.(c) This utility function does have the property that more is preferred to less. However, the marginalrate of substitution is constant, and therefore this utility function does not satisfy the property ofdiminishing marginal rate of substitution.3. (a) Using the formulas in the example from the textbook, one obtains:l = C = (0.75 × 16 − 0.8 − 6)/(1 + 0.75) = 3.89Given the numbers given, we can precisely determine the coordinates of the points in the figureabove: A is (0,6.8), B is (3.89,3.89), D is (9,07,0), with the slope of ABD being − 0.75.30 Williamson • Macroeconomics, Fourth Edition (b) With the new wage, we obtain: l = C = (1.5 × 16 − 0.8 − 6)/(1 + 1.5) = 7.52where A, B and D have the same coordinates as above, and E is (0, 12.8), F is (7.52, 7.52), H is(12.53,0), and the slope of EFH is − 1.5. As there are no substitution effects when goods areperfect complements, the entire move from point B to point F is due to the income effect.4. When the government imposes a proportional tax on wage income, the consumer’s budget constraintis now given by:(1)(),C w t h l T π=−−+−where t is the tax rate on wage income. In the figure below, the budget constraint for t = 0, is FGH.When t > 0, the budget constraint is EGH. The slope of the original budget line is –w , while the slope of the new budget line is −(1 − t )w . Initially the consumer picks the point A on the original budget line. After the tax has been imposed, the consumer picks point B. The substitution effect of the imposition of the tax is to move the consumer from point A to point D on the original indifference curve. The point D is at the tangent point of indifference curve, I 1, with a line segment that is parallel to EG. The pure substitution effect induces the consumer to reduce consumption and increase leisure (work less). T he tax also makes the consumer worse off, in that he or she can no longer be on indifferencecurve, I 1, but must move to the less preferred indifference curve, I 2. This pure income effect moves the consumer to point B, which has less consumption and less leisure than point D, because bothconsumption and leisure are normal goods. The net effect of the tax is to reduce consumption, but the direction of the net effect on leisure is ambiguous. The figure shows the case in which the substitution effect on leisure dominates the income effect. In this case, leisure increases and hours worked fall. Although consumption must fall, hours worked may rise, fall, or remain the same.Chapter 4 Consumer and Firm Behavior: The Work-Leisure Decision and Profit Maximization 315. The budget constraint has a kink due to the tax deduction and is represented in the following figuresby ABDh. Reducing the tax deduction pushes the budget constraint to FEDh.First consider a consumer who does not pay taxes. In the old regime, he would have an optimalbundle somewhere between B and D. Two things can happen. If the bundle is between E and D, there is no change. If it is between B and E, say at H, then the household will reoptimize with the new tax deduction. The new bundle is then either somewhere between E and F, and the MRS equals w(1 −t).Or we obtain a corner solution at E, and the MRS is somewhere between w and w(1 −t). The move from H to E is due to the income effect, and if there is an optimal strictly between E and F, the move from E to that point is due to the substitution effect.32 Williamson • Macroeconomics, Fourth EditionFor a consumer who pays taxes, his wage, and thus is MRS does not change. Thus the move from H to J is a pure negative income effect.6. The increase in dividend income shifts the budget line upward. The reduction in the wage rate flattensthe budget line. One possibility is depicted in the figures below. The original budget constraint HGL shifts to HFE. There are two income effects in this case. The increase in dividend income is a positive income effect. The reduction in the wage rate is a negative income effect. The drawing in the top figure shows the case where these two income effects exactly cancel out. In this case we are left witha pure substitution effect that moves the consumer from point A to point B. Therefore, consumptionfalls and leisure increases. As leisure increases, hours of work must fall. The middle figure shows a case in which the increase in dividend income, the distance GF, is larger and so the income effect is positive. The consumer winds up on a higher indifference curve, leisure unambiguously increases, and consumption may either increase or decrease. The bottom figure shows a case in which theincrease in dividend income, the distance GF, is smaller and so the income effect is negative. The consumer winds up on a lower indifference curve, consumption unambiguously decreases, andleisure may either increase or decrease.Chapter 4 Consumer and Firm Behavior: The Work-Leisure Decision and Profit Maximization 33 7. This problem introduces a higher, overtime wage for hours worked above a threshold, q. Thisproblem also abstracts from any dividend income and taxes.(a) The budget constraint is now EJG in the figure below. The budget constraint is steeper for levelsof leisure less than h − q, because of the higher overtime wage. The figure depicts possiblechoices for two different consumers. Consumer #1 picks point A on her indifference curve, I1.Consumer #2 picks point B on his indifference curve, I2. Consumer #1 chooses to work overtime;consumer #2 does not.(b) The geometry of the figure above makes it clear that it would be very difficult to have anindifference curve tangent to EJG close to point J. In order for this to happen, an indifferencecurve would need to be close to right angled as in the case of pure complement. It is unlikely that consumers wish to consume goods and leisure in fixed proportions, and so points like A and Bare more typical. For any other allowable shape for the indifference curve, it is impossible forpoint J to be chosen.(c) An increase in the overtime wage steepens segment EJ of the budget constraint, but has no effecton the segment JG. For an individual like consumer #2, the increase in the overtime wage has no effect up until the point at which the increase is large enough to shift the individual to a point like point A. Consumer #2 receives no income effect because the income effect arises out of a higher wage rate on inframarginal units of work. An individual like consumer #1 has the traditionalincome and substitution effects of a wage increase. Consumer #1 increases her consumption, but may either increase or reduce hours of work according to whether the income effect outweighsthe substitution effect.8. Lump-sum Tax vs. Proportional Tax. Suppose that we start with a proportional tax. Under theproportional tax the consumer’s budget line is EFH in the figure below. The consumer choosesconsumption, *,C and leisure, *,l at point A on indifference curve I1. A shift to a lump-sum taxsteepens the budget line. The absolute value of the slope of the budget line is (1),− and t has fallent w to zero. The imposition of the lump-sum tax shifts the budget line downward in a parallel fashion. By construction, the lump-sum tax must raise the same amount of revenue as the proportional tax. The consumer must therefore be able to continue to consume *C of the consumption good and *l of leisure after the change in tax collection. Therefore, the new budget line must also pass through point A.The new budget line is labeled LGH in the figure below. With the lump-sum tax, the consumer can34 Williamson • Macroeconomics, Fourth Editiondo better by choosing point B, on the higher indifference curve, I2. Therefore, the consumer is clearly better off. We are also assured that consumption will be greater at point B than at point A, and that leisure will be smaller at point B than at point A.9. Leisure represents all time used for nonmarket activities. If the government is now providing forsome of those, like providing free child care, households will take advantage of such a program,thereby allowing more time for other activities, including market work. Concretely, this translates ina change of preferences for households. For the same amount of consumption, they are now willing towork more, or in other words, they are willing to forego some additional leisure. On the figure below, the new indifference curve is labeled I2. It can cross indifference curve I1 because preferences, as we measure them here, have changed. The equilibrium basket of goods for the household now shifts from A to B. This leads to reduced leisure (from l*1 to l*2), and thus increased hours worked, and increased consumption (from C*1 to C*2) thanks to higher labor income at the fixed wage.Chapter 4 Consumer and Firm Behavior: The Work-Leisure Decision and Profit Maximization 3536 Williamson • Macroeconomics, Fourth Edition 10. The firm chooses its labor input, N d , so as to maximize profits. When there is no tax, profits for thefirm are given by(,).d d zF K N wN π=−That is, profits are the difference between revenue and costs. In the top figure on the following page,the revenue function is (,)d zF K N and the cost function is the straight line, wN d . The firm maximizes profits by choosing the quantity of labor where the slope of the revenue function equals the slope of the cost function:.N MP w =The firm’s demand for labor curve is the marginal product of labor schedule in the bottom figure onthe following page.With a tax that is proportional to the firm’s output, the firm’s profits are given by:(,)(,)(1)(,),d d d d zF K N wN tzF K N t zF K N π=−−=−where the term (1)(,)d t zF K N − is the after-tax revenue function, and as before, wN d is the costfunction. In the top figure below, the tax acts to shift down the revenue function for the firm and reduces the slope of the revenue function. As before, the firm will maximize profits by choosing the quantity of labor input where the slope of the revenue function is equal to the slope of the cost function, but the slope of the revenue function is (1),N t MP − so the firm chooses the quantity oflabor where(1).N t MP w −=In the bottom figure below, the labor demand curve is now (1),N t MP − and the labor demand curvehas shifted down. The tax acts to reduce the after-tax marginal product of labor, and the firm will hire less labor at any given real wage.Chapter 4 Consumer and Firm Behavior: The Work-Leisure Decision and Profit Maximization 37 11. The firm chooses its labor input N d so as to maximize profits. When there is no subsidy, profits forthe firm are given by(,).d d zF K N wN π=−That is, profits are the difference between revenue and costs. In the top figure on the following pagethe revenue function is (,)d zF K N and the cost function is the straight line, wN d . The firm maximizes profits by choosing the quantity of labor where the slope of the revenue function equals the slope of the cost function:.N MP w =The firm’s demand for labor curve is the marginal product of labor schedule in the bottom figurebelow.With an employment subsidy, the firm’s profits are given by:(,)()d d zF K N w s N π=−−where the term (,)d zF K N is the unchanged revenue function, and (w – s )N d is the cost function. Thesubsidy acts to reduce the cost of each unit of labor by the amount of the subsidy, s . In the top figure below, the subsidy acts to shift down the cost function for the firm by reducing its slope. As before, the firm will maximize profits by choosing the quantity of labor input where the slope of the revenue function is equal to the slope of the cost function, (t – s ), so the firm chooses the quantity of labor where.N MP w s =−In the bottom figure below, the labor demand curve is now ,N MP s + and the labor demand curve hasshifted up. The subsidy acts to reduce the marginal cost of labor, and the firm will hire more labor at any given real wage.38 Williamson • Macroeconomics, Fourth Edition 12. Minimum Employment Requirement. Below *,N no output is produced. Thereafter, the productionfunction has its usual properties. Such a production function is reproduced in the first two figures below. At high wages, the firm’s cost curve is entirely above the revenue curve, so the firm hires nolabor, to prevent incurring losses. Only if the wage rate is less than ˆwwill the firms choose to hire anyone. At ˆ,w w= the firm chooses *,N just as it would in the absence of the constraint. Below ˆ,w the labor demand curve is unaffected. The labor demand curve is reproduced in the bottom figure.Chapter 4 Consumer and Firm Behavior: The Work-Leisure Decision and Profit Maximization 39 13. The level of output produced by one worker who works h – l hours is given by(,).s Y zF K h l =−This equation is plotted in the figure below. The slope of this production possibilities frontier is simply .N MP −14. As the firm has to internalize the pollution, it realizes that labor is less effective than it previouslythought. It now needs to hire N (1 + x ) workers where N were previously sufficient. This is qualitatively equivalent to a reduction of z , total factor productivity. The figure below highlights the resulting outcome: the firm now hires fewer people for a given wage and thus its labor demand is reduced.40 Williamson • Macroeconomics, Fourth Edition 15. 0.30.7Y zK n =(a) 0.7.Y n = See the top figure below. The marginal product of labor is positive and diminishing. (b) 0.72.Y n = See the figures below. (c) 0.30.70.72 1.23.Y n n =≈ See the figures below. (d) See the bottom figure below.0.30.30.30.30.31,10.72,1 1.41,220.70.86N N N z K MP n z K MP n z K MP n n −−−−==⇒===⇒===⇒=×≈。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Conjecture 2. Every smooth, closed and oriented 4–manifold is the connected sum of symplectic manifolds, with both the symplectic and the opposite orientations allowed, and of some manifolds with definite intersection forms.
1991 Mathematics Subject Classification. 57R55,57R57,53C15. 1The 4–sphere is the empty connected sum.
1
2
D. KOTSCHICK
This has occurred to several people, especially in the light of the examples constructed in [7], and has been dubbed the current “minimal conjecture” by Taubes. In this note we show that it is false2. Conjecture 1 remains open.
Let d be the order of G. The connected sum decomposition of Y induces a connected sum decomposition X ∼= M #dN, where M is the universal covering of M. As either b+2 (X) ≤ 1 or X is assumed to have a non–trivial Donaldson or Seiberg–Witten invariant, it follows that the intersection form of N is negative definite. By Donaldson’s theorem [1] it is diagonalizable over Z, and therefore either trivial or odd.
2We do not have any proposal for a new “minimal conjecture” in the non–simply connected case.
IRREDUCIBLE FOUR–MANIFOLDS
3
See section 3 for a result in and comments on the case b+2 = 1. For K¨ahler surfaces, Theorem 2 and Corollary 2 could be deduced
If one drops the requirement that X have indefinite intersection form, rational homology spheres give obvious examples.
Recall that a symplectic 4–manifold is called minimal if it contains no symplectically embedded 2–sphere of selfintersection −1. Conjectures 1 and 2 are complementary to Gompf’s conjecture [4] that minimal symplectic 4–manifolds are irreducible. In section 3 we deduce from the recent work of Taubes [11] on the Gromov and Seiberg–Witten invariants that Gompf’s conjecture is true in many cases, including all simply connected manifolds with b+2 > 1. We shall prove:
Conjecture 1. Every smooth, closed, oriented and simply connected 4–manifold is the connected sum of symplectic manifolds, with both the symplectic and the opposite orientations allowed.
When the manifolds under consideration are not simply connected, the situation is more complicated. Then there are obvious counterexamples to Conjecture 1, e.g. rational homology spheres which are not homotopy spheres. Thus, one has to allow definite summands which are more general than nCP or nCP . The natural conjecture is:
Байду номын сангаас
2. Irreducibility of quotient manifolds
Theorem 1 will follow from the following application of the covering trick introduced in [6]:
Proposition 1. Let X be a smooth, closed, simply connected and oriented spin 4–manifold. If b+2 (X) > 1, assume that X has a non–trivial Donaldson or Seiberg–Witten invariant. Suppose a non–trivial finite group G acts freely by orientation–preserving diffeomorphisms of X. Then the quotient Y = X/G is an orientable irreducible 4–manifold.
has no non–trivial finite quotient.
This strengthening of Proposition 1 in [7] goes a long way towards confirming a conjecture made there.
Corollary 2. Minimal symplectic 4–manifolds with b+2 > 1 and with residually finite fundamental groups are irreducible.
This follows from Proposition 1 in the next section.
Corollary 1. There exist orientable irreducible 4–manifolds X with indefinite intersection forms, which are not almost complex (and therefore not complex and not symplectic) with respect to either orientation, and for which the Donaldson and Seiberg–Witten invariants are not defined (or must vanish by definition).
Theorem 2. Let 1. If X ∼= X1#X2
X is
be a minimal symplectic a smooth connected sum
4–manifold with b+2 (X) > decomposition of X, then
one of the Xi is an integral homology sphere whose fundamental group
We will show:
Theorem 1. There exist oriented irreducible 4–manifolds X with indefinite intersection forms and with π1(X) = Z and b+2 (X) ≡ b−2 (X) ≡ 0 (mod 2).
Recall the following definition:
Definition 1. A smooth closed 4–manifold X is irreducible if for every smooth connected sum decomposition X ∼= X1#X2 one of the summands Xi must be a homotopy sphere.
easily from the reduction of the Seiberg–Witten equation to the K¨ahler vortex equation and the study of effective divisors on complex surfaces, due to Kronheimer–Mrowka and Witten [13].
