Superconductivity controlled by the magnetic state of ferromagnetic nanoparticles
“第17届凝聚态理论与统计物理学术会议”日程(初稿)

吴超(西安交通大学) 题目: The influence of local arrangements of oxygen adatoms on the energetics of O2 dissociation over Pt(111) 赵明文(山东大学) 题目: 新型碳材料结构设计和性能调控的理 论模型 李希茂(北京宏剑公司)(12:10-12:25) 题目: 第一原理计算材料的缺陷和掺杂特性
李文飞(南京大学) 题目: 蛋白质分子体系多尺度理论模拟
孙久勋(电子科技大学) 题目: Improvement of unified mobility model and electrical properties for organic diodes under dc and ac conditions
关丽(河北大学):Structural stability and electronic properties of two nonstoichiometric SrTiO3 phases
休息
报告厅 3(主题: 冷原子物理) 分会报告 ST3.3 主席:成 泽 教授(华中科技大学) (邀请报告) 周琦(香港中文大学) 题目:自旋轨道耦合下波色凝聚体的命运
主席:金国钧 教授(南京大学)
(邀请报告) 杨义峰(中国科学院物理研究所) (邀请报告) 孟胜(中国科学院物理研究所)
题目:重费米子物理中的演生现象
题目:Energy Conversion At Nanoscale
Topological defects in triplet superconductors UPt$_{3}$, Sr$_{2}$RuO$_{4}$, etc

2
FIG. 2: Phase diagram of U1−xThxBe13 from Heffner et al [41].
the specific heat data [11]. Also as discussed elsewhere [27, 28], the two gap model is of little help in this matter. More recently the quasiparticle density of states in the vortex state in Sr2RuO4 has been reported [29]. Indeed the
observed quasiparticle density of states is very consistent with that predicted for an f-wave order parameter [30]. Also many of these superconductors are triplet: UPt3, Sr2RuO4, (TMTSF)2PF6, U1−xThxBe13, URu2Si2, PrOs4Sb12, UNi2Al3 and CePt3Si, for example.
After a brief introduction on nodal superconductors, we review the topological defects in triplet
superconductors such as UPt3, Sr2RuO4, etc. This is in part motivated by the surprising discovery of
Since 2001 Izawa et al have determined the gap function ∆(k) in Sr2RuO4[18],CeCoIn5 [19], κ−(ET)2Cu(NCS)2 [20], YNi2B2C [21], and PrOs4Sb12 [22, 23] via the angle-dependent magnetothermal conductivity. These |∆(k)|’s are shown in Figure 1. In addition, the gap function of UPt3 was established around 1994-6 as E2u through the anisotropy in the thermal conductivity [24] and the constancy of the Knight shift in NMR [25]. Somewhat surprisingly all these superconductors are nodal and their quasiparticle density of states increases linearly in |E| for |E|/∆ ≪ 1:
iDrive TM lite LED驱动器产品简介说明书

Product OverviewThe powerful new i Drive TM lite LED driver is designed to optimize the performance of high power lighting fixtures using high power LEDs including Luxeon TM.The patented i Drive TM lite technology enables excellent colour matching and 100% smooth dimming with precise DC current control combined with advanced automatic heat management system to enhance the long life of both fixtures and LED boards. The 55 Watt system provides a universal voltage input with both UL and CE approvals so you can install them in practically any location.The i Drive TM lite has been designed to make installation simple and to save time by using standard power and DMX connectors with a unique user interface to control all i Drive TM lite functions. There are no complicated DIP switches!The patented thermal control of attached LED boards, using our unique Colour Cool TM Technology, optimises your LED installation for any environment.i Drive TM lite can be controlled by DMX512, or use the hundreds of pre-programmed settings to provide independent scenes, colour combinations and effects.Features•Compact size and rugged construction with standard5-pin XLR DMX in/out connectors.•Universal voltage input with standard IEC connector.•Patented Colour Cool TM thermal management system to optimise and prolong the life of fixtures and LEDs.•The i Drive TM lite technology is licenced and patented in the UK and USA with Worldwide applications pending.•Patented colour mixing 3 channel system.•Simple 3 rotary switch interface sets DMX address and controls all additional pre-set functions.•Smooth dimming control 0 - 100%.•High efficiency (>88%).•Long life and high reliability (50,000 hours).•LED lamp connection with 8 pin RJ45 connector.•Short and open circuit protection.•Standalone mode (no DMX controller required) incorporating many static and dynamic colour functions and programmes.•Self test functions.•No binning of LEDs results in cost savings.•Internal Thermal Protection.•CE ApprovedU S E R M A N U A LThe i Drive lite is one of afamily of devices specificallydesigned for the control anddimming of LED Fixtures.Mains IndicatorWiring Fault IndicatorDMX Indicator Welcome to the iDrive lite , with a host of built in features and protection for your LED fixtures.The iDrive lite is designed to control fixtures containing between 18 and 36 RGB LED's.Please ensure that the LED fixture is plugged into the iDrive RJ45 connector before the mains is switched on, this is important since the system will perform a diagnostic scan of the LED fixture when powered up.The diagnostic scan will test for two functions.1.Open or short circuits in the LED fixture and wiring. If this is detected the faulty channel will be isolated. The RED LED 'wiring fault indicator' will illuminate to confirm this. The iDrive should be turned off at the mains and the fault rectified before powering up the system again.2. The second scan will look for a thermistor on the LED fixture, as recommended in the 'wiring specification' (page 4). If a thermistor is found the 'thermal feedback protection’will be activated in the iDrive.Both these scans take less than 1 second to perform and only take place on initial power up of the system.The iDrive can be used in DMX mode or standalone mode.For DMX SettingsThe rotary switches should be set to between 001and 510. Normally address 0.0.1 is sufficient for a 3channel and master DMX controller.For Stand Alone SettingsThe iDrive contains many pre-set programmes.600 - 636- This setting provides 36 different preset colours - 636 being a white setting, i.e. all LEDS full on.700 - 799- These are the cross fade settings with different speed functions.800 - 819 - Cycle Wash Pre-set.There are two preset cyclic washes, eitherclockwise or anti-clockwise with speed controlMains Indicator - Indicates power onto the iDriveDMX Indicator - When the rotary switches are set to a DMX address i.e. between 001 and 510,this indicator will flash until the iDrive receives a DMX input via the DMX 5-pin XLR input.Once a DMX signal is received, the amber indicator stops flashing and stays permanently on.Wiring Fault Indicator - The iDrive hasshort/open circuit protection. In the event of the LED fixture being incorrectly wired, the indicator will be permanently on until the fault in the LED fixture has been corrected.The iDrive uses DMX 512A - the latest ESTA DMX standard, using isolated 5-pin XLR connections forboth input and output.The iDrive can be networked from one single DMX inputx100x10x10 - 90 - 90 - 9Cross fade settings between colours700 - 790Speed Settings 0 = Fastest9 = Slowestx100x10x10 - 90 - 90 - 9Cycle Wash pre-sets either 800 - 810Speed Settings 0 = Fastest9 = SlowestDMX AND PRE-SET PROGRAMME SETTINGSSwitch Settings Function001 - 510DMX-512A start address 600 - 636Fixed Colour pre-set 700 - 799Cross Fade pre-set 800 - 819Cyclic Wash pre-set0 - 90 - 90 - 9TM DMX IN OUTTM DMX IN OUTTMDMX IN OUTDMX InputTERMINATORWiring configurations for 5-pin XLR G (ground cable shield) to XLR pin No. 1- (negative) to XLR pin No. 2+ (positive) to XLR pin No. 3DMX TerminationIn accordance with good practice of DMX cabling networks. (ESTA & USITT). It is recommended that the last DMX output plug is terminated correctly by fitting a 120 Ohm resistor across terminals 2 & 3 as shown.61-234+12 0 RTerminate with a metal-film resistor of 120 [Ohm]Solder side: male18 x RGB systems12 x RGB systemsTypical wiring configurations for 350mA LED RGB systemWIRING SPECIFICATION INFORMATIONRJ45 WIRING INPUT 1 = Red +2 = Red -3 = Green +4 = Green -5 = Blue +6 = Blue -7 = Thermistor Ground*8 = LED Temperature** IST Ltd recommend that a 10K ohm SMT thermistor type: EPCOS B57621C103J62 is located in the centre ofthe LED board foreffective thermal management control.SPECIFICATIONSELECTRICAL CHARACTERISTICSInputInput Voltage Range : 100 - 240V AC Input Frequency : 50 - 60 Hz Power Consumption : 6 - 55 W Power Power Factor : 0.95Efficiency : 88%Connection: standard IEC Insulation Class: OneOutputPower Output Range : 0 - 16.8 W Per ChannelMaximum Output Current : 350mA @ 100% Maximum Output Voltage : 14V - 48V DC Connection: RJ45 (8 pin)Control Input Dimming Control : DMX-512AConnection: standard XLR 5 pin Dimming Range: 0 - 100 %DMX Start Address Range : 1 - 510 via 3 rotary BCD switches.Mechanical Mounting : Four 3mm holes for wall fixing.Construction : Aluminum casing for improved thermal performance.Weight:600 gramsEnvironmentalOperating Ambient Temperature : -20ºC to + 50°C Storage Ambient Temperature : -20ºC to + 70ºC Case Temperature : + 65ºC Relative Humidity: 80%Lifetime (failures after 50,000 hours): 5%DimensionsThermal Protection:To protect the components used in the production of the iDrive, a thermal over-load protection system has been built into the circuit.Should the ambient temperature, inside the iDrive casing exceed 65º centigrade, the thermal protection system will be activated and the iDrive will be switched off.Once the internal temperature falls to a normal operating level the iDrive will automatically switch itself back on.Warranty and Returns Policy:Product warranty or service will not be honored if:1.The product has been repaired, modified or altered2.The serial number is defaced or missing3.Operation of the product has occurred outside of the published environmental specification.Should the iDrive fail in service within 12 months from the purchase date, please return the unit to your supplier for replacement.There are no serviceable parts in the iDrive,opening of the unit will void all warranties.。
Superconductivity-induced changes of the phonon resonances in RBa_2Cu_3O_7 (R=rare earth)

a r X i v :c o n d -m a t /0101217v 1 [c o n d -m a t .s u p r -c o n ] 15 J a n 2001Superconductivity-induced changes of the phonon resonances in R Ba 2Cu 3O 7(R =rare earth)S.Ostertun,J.Kiltz,A.Bock ∗,and U.MerktInstitut f¨u r Angewandte Physik und Zentrum f¨u r Mikrostrukturforschung,Universit¨a t Hamburg,Jungiusstraße 11,D-20355Hamburg,GermanyT.WolfInstitut f¨u r Festk¨o rperphysik,Forschungszentrum Karlsruhe,D-76021Karlsruhe,Germany(February 1,2008)We observe a characteristic energy ¯h ωc ≈2.1eV which separates regions of different behavior of the phonon intensities in the Raman spectra of the R Ba 2Cu 3O 7system.A superconductivity-induced drop of phonon intensities is found for the oxygen modes O(4)and O(2)-O(3)only for excitation energies below ¯h ωc .This intensity drop indicates an order parameter which affects energies in the vicinity of ¯h ωc .PACS numbers:74.25.Gz,74.25.Jb,74.25.Kc,74.72.Bk,78.30.ErI.INTRODUCTIONSeveral superconductivity-induced changes of pa-rameters describing the phonons in the cuprate su-perconductors can be determined by Raman spec-troscopy.Frequency and linewidth anomalies like hard-ening and broadening have been the subject of severalexperimental 1–3and theoretical 4–7studies.While the superconductivity-induced phonon self-energy effects al-low conclusions regarding the order parameter only for energies similar to the phonon energies (up to 100meV),high-energy (>1eV)questions cannot be addressed this way.Resonant Raman scattering is an experimental tech-nique which allows one to examine the physics at high energy through the resonances of phonons.In contrast to reflectivity or transmission spectroscopy the affected phonons provide direct information on incorporated elec-tronic bands as the assignments of the phonon modes in the spectra to the vibrating atoms is well known through group theoretical calculations and isotope substitution experiments.Thus,the dependence of the phonon inten-sities on temperature and excitation energy can provide information about the scattering mechanism and espe-cially about the superconducting state.In contrast to the usually observed increase of the intensity 8,9below the critical temperature T c we observe a drop of intensity in several modes when exciting with photons of energy ¯h ωi <2.1eV.In the same region of excitation energy the apical oxygen mode at 500cm −1shows a violation of the symmetry selection rule which cannot be explained in terms of an orthorhombic distor-tion.Summarizing our data we conclude that the origin of the intensity anomaly is related to a modification of the Cu-O charge transfer mechanism in the superconducting state in comparison to the normal state.II.EXPERIMENTAL DETAILSSubject of this paper are Yb-123,Er-123,Sm-123and Nd-123single crystals,with T c =76K,81K,94K,and 90K,respectively.All measured single crystals were grown with a self-flux method and annealed with oxygen under high pressure.10Due to the high oxygen content and the different radii of the rare earth atoms Yb-123and Er-123are overdoped whereas Sm-123and Nd-123are nearly optimally doped.11The laser beam is focused onto the sample along the c-direction.The orthorhombic symmetry of the R -123system can be treated as tetrag-onal.Accordingly,the Raman active phonons are of A 1g and B 1g symmetry,which are allowed for z(xx)¯z /z(x ′x ′)¯zand z(xx)¯z /z(x ′y ′)¯z polarizations in the Porto notation,respectively.All polarizations are specified with respect to the axes along the Cu-O bonds of the CuO 2planes,primed polarizations are rotated by 45o .For simplicity z and ¯z are omitted in the following.The measurements were performed using several lines of Ar +,He-Ne and Ti:Saphir lasers in quasi-backscattering geometry.The details of the setup are described elsewhere.12In order to achieve a high accuracy of intensity measurements the laser power was monitored during the measurements.All spectra were corrected for the response of the detector and the optical system.Also,they are normalized to the incident photon rate.For a comparison of intensities obtained with different excitation energies the cross-section is calculated from the efficiencies using ellipsometric data of Yb-123.The complex dielectric function of Bi-2212shows only a slight variation with temperature 13at our excitation energies between 1.68eV and 2.71eV and the resulting reflectivity exhibits maximal variations below 1%.As the reflectivity of the R -123system behaves similar,14Raman spectra of all temperatures can be evaluated with the ellipsometric data obtained at room-temperature.In Fig.1the com-plex dielectric function of Yb-123at room-temperature is given.From the real part ε1of the dielectric functionwe can estimate a screened plasma frequencyωp of1.44 eV.The low-temperature background intensity of the cross-section atω≈700cm−1is plotted versus the exci-tation energy for the x’x’and x’y’polarization geometry in the inset of Fig.1in the top and bottom panel,re-spectively.Within experimental error no significant res-onance of the cross-section can be determined.The scat-tering of the data points results from uncertainties in the adjustment of the setup.Thus,the measured phonon intensities will scatter in a similar way.To obtain the integrated phonon intensities wefitted the spectra to the model presented in a previous paper.3An extended Fano profile is described byI(ω)=CC2−2ǫ(ω)R∗(ω)̺∗(ω)C2 (1)with the substitutions̺∗(ω)=Cg2σ̺eσ(ω),R∗(ω)= Cg2σR eσ(ω)+R0,ǫ(ω)= ω2−ω2ν(ω) /2ωpγ(ω),γ(ω)=Γ+̺∗(ω)/C,andω2ν(ω)=ω2p−2ωp R∗(ω)/C.Here,̺eσ(ω)and R eσ(ω)are the imaginary and real part of the electronic response function,respectively,and gσis the lowest-order expansion coefficient of the electron-phonon vertex.The bare frequency and linewidth of the phonon areωp andΓ,respectively.The constant C is a scaling parameter due to the use of arbitrary units in the spectra. We use this extended Fano profile for the asymmetric phonon modes and Lorentzian profiles for the remaining phonons.As described in Ref.3,the measured electronic response̺∗(ω)is described via a phenomenological for-mula which contains a tanh(ω/ωT)term for the incoher-ent background and two coupled Lorentzian profiles for the redistribution of the spectra below T c—one for the pair breaking peak and a negative one for the suppres-sion of spectral weight at low Raman shifts.This formula allows a simultaneous description of R∗(ω).III.RESULTSIn the top panels of Fig.2we present spectra of Yb-123 obtained at15K nominal temperature in x’x’(A1g+B2g) and in x’y’(B1g)geometry with excitation energies of 2.71eV and1.96eV.The original spectra have been scaled to equal background intensity for clarity.Exciting with2.71eV all phonons show up mainly in the spec-tra of the expected polarization geometry.For the x’x’geometry we have the Ba mode at120cm−1,Cu(2)at 150cm−1,O(2)+O(3)at440cm−1,and O(4)at500 cm−1.For the x’y’geometry the O(2)-O(3)mode ap-pears at340cm−1.In contrast,exciting with1.96eV the O(4)mode has nearly vanished in x’x’geometry but only slightly changed in x’y’symmetry.Nearly the same behavior is observed for the resonance of the intensity of the O(4)mode in Er-123,Sm-123,and Nd-123.Or-thorhombic distortion,which is usually mentioned as the cause for phonon intensity in forbidden symmetries,can-not explain this behavior.Though resonance effects can disturb symmetry selection rules in principle,the symme-try violation should appear under resonance conditions but not out of resonance.Subtracting the phononic contribution we get the elec-tronic background of Yb-123,which is plotted in the lower panels of Fig.2.The x’x’spectra are nearly identi-cal for both excitation energies and even the x’y’spectra are quite similar.The B1g pair breaking peak lies at a relatively low energy of320cm−1due to the high doping level15of p≈0.2,which is determined from T c/T c,max (see Ref.16).There is no enhancement of the gap exci-tation from1.96eV to2.71eV.This is consistent with the behavior observed for Bi-2212which shows non-resonant gap excitations for doping levels up to approximately0.2 and a gap resonance only for higher doping levels as re-ported in Ref.17.Figure3depicts the results of a detailed study of the integrated O(4)mode intensity.For temperatures above T c the x’x’intensity increases when the temperature is lowered for excitation energies of1.96eV,2.41eV,and 2.71eV.This increase is hardly influenced by the phase transition below T c for2.41eV and2.71eV in contrast to 1.96eV where the intensity suddenly drops.The compar-atively high values for intensities of the2.71eV data in the range of100K to200K are due to the scattering men-tioned in the introduction.Here it results from a slight shift of the laser spot with respect to the entrance slit of the spectrometer.On the other hand the x’y’intensity shows the same behavior for all measured excitation en-ergies:a slight increase with decreasing temperature not or only slightly affected by T c.For temperatures above T c the relation for the x’x’intensities I2.71:I2.41:I1.96is approximately5.0:3.1:1.0as indicated by the solid lines in the left panel of Fig.3.The superconductivity-induced drop of the x’x’intensity at low excitation energies leads to a superconductivity-induced enhancement of the res-onance profile of the x’x’O(4)mode intensity.Figure4yields the excitation energy¯hωc=2.1±0.1cm−1where the O(4)mode exhibits equal intensity in x’x’and x’y’geometries.This is also the energy of the crossover between the superconductivity-induced in-crease and decrease of the x’x’intensity shown in Fig.3, i.e.2.0-2.4eV.While the measurements have been car-ried out in this detail for Yb-123only,spectra of Er-123, Sm-123,and Nd-123at low temperature exhibit a simi-lar behavior as shown in the inset of Fig.4.There the ratio of the x’x’and x’y’O(4)mode intensity is plotted against the excitation energy.It exhibits no significant doping dependence.The O(2)-O(3)mode displays a sim-ilar behavior in the x’y’as the O(4)mode in the x’x’symmetry.In Fig.5the temperature dependence of its intensity is plotted for excitation energies¯hωi=1.68eV, 1.96eV,2.41eV,and2.71eV.Above T c the intensity is only slightly affected by the temperature,below T c theintensity drops only for excitation energies below¯hωc. The intensity of the Cu(2)mode shows a strong in-crease with decreasing temperature above T c but also a slight drop below T c.The intensity at low tempera-tures is approximately70%–80%of the highest value at T c.While this ratio is independent of excitation energy and polarization geometry the resonance energy itself de-pends on the polarization geometry as shown in Fig.6. Gaussian profiles guide the eye and help to determine the resonance energy of the Cu(2)mode intensity,which is about2.1eV for x’x’and well below2.0eV for x’y’sym-metry.The Ba mode which is not shown here exhibits a slight intensity gain below T c,which seems to be unaf-fected by¯hωc.The x’x’intensity is resonant at2.6eV or even higher energy,the x’y’intensity follows this profile with about30%of the intensity in x’x’geometry.IV.DISCUSSION AND CONCLUSIONS Summarizing our data wefind a characteristic energy ¯hωc≈2.1eV which separates the regions of red and blue excitations.It is very similar to the crossing point at 2.2eV observed in the imaginary part of the dielectric function13of Bi-2212.For red excitation the R-123sys-tem exhibits a superconductivity-induced drop of the in-tensity of the O(4)mode in x’x’and the O(2)-O(3)mode in x’y’symmetry,for blue excitation the drop is absent. Additionally,for red excitations the symmetry selection rule of the O(4)mode is violated as the x’y’intensity exceeds the x’x’intensity.The maximum of the inten-sity of the Cu(2)mode in x’x’symmetry coincides with ¯hωc.All these effects are characterized by¯hωc indicating a common microscopic origin.Low temperature mea-surements of overdoped Er-123as well as of underdoped Sm-123and Nd-123show a similar behavior as Yb-123, especially the symmetry violation of the O(4)mode for excitation energies below¯hωc.Thus¯hωc could be a gen-eral property of the R-123system without a significant doping dependence.Friedl et al.18observed a superconductivity-induced gain of the xx phonon intensities of Y-123films on SrTiO3 substrates,which are independent of excitation energy in the range of1.92eV to2.60eV,a drop of intensity is ob-served only for the Cu(2)mode.The differences between their data and the data presented here may in princi-ple result from the different doping levels of Y-123and Yb-123.But as we have noted above,the effects are mainly independent of doping,our explanation for the discrepancies between Friedl’s and our data is the fol-lowing:As the lattice constant of Y-123(3.82/3.88˚A) is smaller than that of SrTiO3(3.91˚A)and as the ther-mal expansion coefficient of Y-123(11.7˚A/K)exceeds that of SrTiO3(9.4˚A/K),the lattice mismatch increases with decreasing temperature.The additional stress may explain the high intensity increase I(100K):I(250K)≈2.5 of the Y-123films in comparison to I(100K):I(300K)≈1.3of the Yb-123single crystal.Thus,the drop below T c is masked in this strong increase,especially as Friedl et al. recorded xx+yy data which include x’y’where no inten-sity drop appears.In underdoped R-123the O(2)-O(3) mode is known to show a strong intensity gain.3Thus, the intensity drop of the O(2)-O(3)mode can only be observed in overdoped samples as the drop is not masked by an intensity gain.Sherman et al.8explained the increase of the total B1g mode intensity below T c of Y-123in terms of an exten-sion of the number of intermediate electronic states near the Fermi surface that participate in the Raman process. Accordingly,the doping dependence of the gain of the O(2)-O(3)mode intensity can be explained in this pic-ture by the vicinity to the Fermi-level.Analogously,an intensity drop means a reduction of the number of inter-mediate states which is not consistent with this model. Possibly the intensity gain and the intensity drop have different origins,which are doping dependent and inde-pendent,respectively.Due to the different resonance profiles in x’x’and x’y’geometry the drop of the phonon intensities can only be explained in the framework of a resonant theory which must account for the bands of the initial andfinal states. Calculations for the phonons of Y-123in the supercon-ducting state which have been performed by Heyen et al.19and which are based on the local-density approxi-mation and the linear muffin-tin-orbital method do not show any signature of an intensity drop around2eV for xx/yy and zz symmetry.The calculated xx/yy intensity of the O(4)mode has a local intensity minimum at2.0 eV but at1.6eV the predicted intensity is more than twice the intensity at2.0eV excitation energy which is strong contrast to our data.As no appropriate theory is available we try an in-terpretation in a simple picture:The intensity drop is observed for the Cu(2)mode and,for excitation energies below¯hωc,for the plane-related O(2)-O(3)mode and for the x’x’O(4)mode.This indicates that plane bands are responsible for the observed resonance effect.In case of the O(4)mode two processes seem to contribute to the phonon intensity,as the strongly resonant x’x’intensity exhibits the drop below¯hωc and the x’y’intensity shows no resonance and no superconductivity-induced effects. As the characteristic energy¯hωc which separates the re-gions with and without the superconductivity-induced in-tensity drop is the same for the O(4)mode as for the O(2)-O(3)mode,we conclude that the relevant initial or final state bands are the same.Thus,we attribute the x’x’intensity to a process involving that plane bands.On the other hand the x’y’intensity is attributed to the chain bands.Another hint for this interpretation is that the pairing mechanism is believed to be located in the CuO2 planes.Thus,superconductivity-induced effects would be expected for processes which involve plane bands. Within a simple band structure picture the opening of the gap cannot explain the threshold of¯hωc directly:If the opening of the gap would enhance the energy distancebetween the possible initial and final states for an allowed transition to a value which exceeds the excitation energy,this process would also be suppressed for lower excitationenergiesin thenormal state.Figure 5clearly shows that the behavior of the phonon intensity for 1.96eV and 1.68eV excitations is essentially the same.Thus,only a fun-damental change of the band structure could explain the different behavior for blue and red excitation.As the x’x’intensity of the Cu(2)mode has its maximum right at the critical energy ¯h ωc (see Fig.6)the fundamental change with the superconducting transition should take place in a plane band involving the Cu(2)atom.As mentioned above the O(4)mode intensity in x’x’and x’y’symme-try results from different processes due to the coupling to the Cu(2)-O(2)-O(3)plane bands and to the Cu(1)-O(1)chain bands,respectively.In comparison to the superconducting gap 2∆the critical energy ¯h ωc is quite a large energy.Superconductivity-induced high-energy effects have been observed previously by thermal difference reflectance (TDR)spectroscopy 14and by ellipsometry.13In the el-lipsometry data a crossing point in ε2at 2eV separates two spectral regions of different behavior in their tem-perature dependencies.The TDR spectra of Y-123and other high-temperature superconductors exhibit a devi-ation from unity in the ratio R S /R N of the supercon-ducting to normal state spectra at high photon ener-gies (≈2.0eV).Within the Eliashberg theory the devia-tion can only be explained if the electron-boson coupling function contains a high-energy component in addition to the electron-phonon interaction leading to an order parameter which is non-zero for similar energies.The microscopic origin of the high-energy interaction is most likely a d 9−d 10L∗Present Address:Basler AG,An der Strusbek 60-62,D-22926Ahrensburg,Germany.1E.Altendorf,X.K.Chen,J.C.Irwin,R.Liang,and W.N.Hardy,Phys.Rev.B 47,8140,1993.2V.G.Hadjiev,Xingjiang Zhou,T.Strohm,M.Cardona,Q.M.Lin,and C.W.Chu,Phys.Rev.B 58,1043,1998.3A.Bock,S.Ostertun,R.Das Sharma,M.R¨u bhausen,and K.-O.Subke,Phys.Rev.B 60,3532,1999.4R.Zeyher and G.Zwicknagel,Z.Phys.B 78,175,1990.5E.J.Nicol,C.Jiang,and J.P.Carbotte,Phys.Rev.B 47,8131,1993.6T.P.Devereaux,Phys.Rev.B 50,10287,1994.7B.Normand,H.Kohno,and H.Fukuyama,Phys.Rev.B 53,856,1996.8E.Ya.Sherman,R.Li,and R.Feile,Phys.Rev.B 52,R15757,1995.9O.V.Misochko,E.Ya.Sherman,N.Umesaki,K.Sakai,and S.Nakashima,Phys.Rev.B 59,11495,1999.10T.Wolf,W.Goldacker,B.Obst,G.Roth,and R.Flukiger,J.Crystal Growth 96,1010,1989.11Y.Xu and W.Guan,Phys.Rev.B 45,3176,1992.12M.R¨u bhausen,C.T.Rieck,N.Dieckmann,K.-O.Subke,A.Bock,and U.Merkt,Phys.Rev.B 56,14797,1997.13M.R¨u bhausen,A.Gozar,M.V.Klein,P.Guptasarma,and D.G.Hinks,submitted to Phys.Rev.B 14M.J.Holcomb,C.L.Perry,J.P.Collman,and W.A.Little,Phys.Rev.B 52,6734,1996.15A.Bock,Ann.Phys.8,441,1999.16J.L.Tallon,C.Bernhard,H.Shaked,R.L.Hitterman,and J.D.Jorgensen,Phys.Rev.B51,12911,1995.17M.R¨u bhausen,O.A.Hammerstein,A.Bock,U.Merkt, C.T.Rieck,P.Guptasarma,D.G.Hinks,and M.V.Klein, Phys.Rev.Lett.82,5349,1999.18B.Friedl,C.Thomsen,H.-U.Habermeier,and M.Cardona, Solid State Commun.78,291,1991.19E.T.Heyen,S.N.Rashkeev,I.I.Mazin,O.K.Andersen, R.Liu,M.Cardona,and O.Jepsen,Phys.Rev.Lett.65, 3048,1990.d ie l e c t r i cf u n c t i o nenergy (eV)plex dielectric function ε1+i ε2of Yb-123at room temperature.The inset depicts the Raman cross-section for the x’x’(top panel)and the x’y’(bottom panel)polariza-tion symmetries.Lines mark the average values.6002000Raman shift (cm -1)i n t e n s i t y (a r b . u n i t s )FIG.2.Raman spectra of Yb-123in x’x’and x’y’polariza-tion geometries taken with excitation energies ¯h ωi =2.71eV (dashed lines)and 1.96eV (solid)at 15K cryostat tempera-ture.The lower panels show the background spectra obtained by subtraction of thephonons.O (4) m o d e i n t e n s i t y (a r b . u n i t s )temperature (K)FIG.3.Temperature dependence of the integrated O(4)Raman intensity in Yb-123in x’x’(closed symbols)and x’y’(open symbols)polarizations for excitation energies 2.71eV (triangles),2.41eV (diamonds),1.96eV (circles),and 1.68eV (squares).Solid lines serve as guides to the eye,dashed lines indicate T c .The x’y’data are offset as indicated.O (4) m o d e i n t e n s i t y (a r b . u n i t s )energy (eV)FIG.4.O(4)intensity of Yb-123at 15K in x’x’(closed symbols)and x’y’(open symbols)polarization geometry.Lines are guides to the eye.The inset shows the ratio of the x’x’and x’y’intensity at 15K for various R -123single crystals that correspond to different doping levels.p h o n o n i n t e n s i t y (a r b . u n i t s )temperature (K)FIG.5.Temperature dependence of the O(2)-O(3)inten-sity of Yb-123for excitation energies ¯h ωi =2.71eV (tri-angles),2.41eV (diamonds),1.96eV (circles),and 1.68eV (squares).The dotted line indicates T c .x 'x ' p h o n o n i n t e n s i t yexcitation energy (eV)x 'y ' p h o n o n i n t e n s i t yFIG.6.Resonance of the intensity of the Cu(2)mode in Yb-123at 15K in x’x’(closed symbols)and x’y’(open sym-bols)geometry.Dotted Gaussian profiles guide the eye.。
Supercapacitor

SupercapacitorSupercapacitors (SC),[1]comprise a family of electrochemical capacitors. Supercapacitors, sometimes called ultracapacitors or electric double-layer capacitor (EDLC), don't have a conventional solid dielectric. The capacitance value of an electrochemical capacitor is determined by the combination of two storage effects:[2][3][4]∙Double-layer capacitance–electrostatic storage of the electrical energyachieved by separation of charge in a Helmholtz double layer at the interfacebetween the surface of a conductive electrode and a electrolyte. The separation of charge distance in a SC is on the order of a few Angstroms (0.3–0.8 nm) and is static.[5]∙Pseudocapacitance–Electrochemical storage of the electrical energy, achieved by redox reactions with specifically adsorbed ions from the electrolyte, intercalation of atoms in the layer lattice or electro-sorption, underpotential deposition of hydrogen or metal adatoms in surface lattice sites which result in a reversible faradaic charge-transfer.[5]The ratio of the storage resulting from each principle can vary greatly, depending on electrode design and electrolyte composition. Pseudo-capacitance can increase the capacitance value by as much as an order of magnitude over that of the double-layer by itself.[1]Supercapacitors are divided into three families, based on the design of the electrodes: ∙Double-layer capacitors –with carbon electrodes or derivates with muchhigher static double-layer capacitance than the faradaic pseudocapacitance ∙Pseudocapacitors –with electrodes out of metal oxides or conducting polymers with a high amount of faradaic pseudocapacitance∙Hybrid capacitors – capacitors with special electrodes that exhibit significant capacitance from both principlesHierarchical classification of supercapacitors and related typesSupercapacitors occupy the gap between traditional capacitors and rechargeable batteries. They have higher capacitance values per unit volume and greater energy density than other capacitors. They support up to 12 Farads/1.2 Volt, with capacitance values up to 10 times that of electrolytic capacitors.[1] While existing supercapacitors have energy densities that are approximately 10% of a conventional battery, their power density is generally 10 to 100 times greater. Power density is defined as the product of energy density, multiplied by the speed at which the energy is delivered to the load. The greater power density results in much shorter charge/discharge cycles than a battery is capable, and a greater tolerance for numerous charge/discharge cycles.Within electrochemical capacitors, the electrolyte is the conductive connection between the two electrodes, distinguishing them from electrolytic capacitors, in which the electrolyte is the cathode and second electrode.Supercapacitors are polarized and must operate with correct polarity. Polarity is controlled by design with asymmetric electrodes, or, for symmetric electrodes, by a potential applied during the manufacturing process.Supercapacitors support a broad spectrum of applications for power and energy requirements, including:[6]∙Long duration, low current, for memory backup in (SRAMs)∙Power electronics that require very short, high current, as in the KERSsystem in Formula 1 cars∙Recovery of braking energy for vehiclesHistoryDevelopment of electrochemical capacitorsIn the early 1950s, General Electric engineers began experimenting with devices using porous carbon electrodes for fuel cells and rechargeable batteries. Activated charcoal is an electrical conductor that is extremely porous carbon with a high specific surface area, providing a useful electrode material. In 1957 H. Becker developed a "Low voltage electrolytic capacitor with porous carbon electrodes".[7][8][9] He believed that the energy was stored as a charge in the carbon pores as in the etched foils of electrolytic capacitors. Because the double layer mechanism was not known at the time, he wrote in the patent: "It is not known exactly what is taking place in the component if it is used for energy storage, but it leads to an extremely high capacity." General Electric did not immediately pursue this work.In 1966 researchers at Standard Oil of Ohio (SOHIO) developed another version of the devices as ―Electrical energy storage apparatus‖, while working on experimental fuel cell designs.[10][11]The nature of electrochemical energy storage was not described in this patent. Even in 1970, the electrochemical capacitor patented by Donald L. Boos was registered as an electrolytic capacitor with activated carbon electrodes.[12]Principle construction of a supercapacitor; 1. power source, 2. collector, 3.polarized electrode, 4. Helmholtz double layer, 5. electrolyte having positive and negative ions, 6. Separator. By applying a voltage to the capacitor at both electrodes a respective Helmholtz double layer is formed, which has a positive or negative layer of ions from the electrolyte deposited in a mirror image on the respective opposite electrode.These early electrochemical capacitors used a cell design of two aluminum foils covered with activated carbon - the electrodes - which were soaked in an electrolyte and separated by a thin porous insulator. This design gave a capacitor with a capacitance value in the one "farad" area, which was significantly higher than for electrolytic capacitors of the same dimensions. This basic mechanical design remains the basis of most electrochemical capacitors.SOHIO did not commercialize their invention, licensing the technology to NEC, who finally marketed the results as ―supercapacitors‖ in 1971, to provide backup power for computer memory.[11] Other manufacturers followed from the end of the 1970s. Around 1978 Panasonic marketed its "Goldcaps‖ brand.[13]This product became a successful back-up energy source for memory backup applications.[11]The competition started some years later. In 1987 ELNA"Dynacap"s entered the market.[14] This generation had relatively high internal resistance, which limited the discharge current. They were used for low current applications like powering SRAM chips or for data backup.At the end of the 1980s improved electrode materials led to higher capacitance values and in lower resistance electrolytes that lowered the ESR in order to increase the charge/discharge currents. This led to rapidly improving performance and a rapid reduction in cost.The first supercapacitor with low internal resistance was developed in 1982 for military applications through the Pinnacle Research Institute(PRI), and were marketed under the brand name "PRI Ultracapacitor". In 1992, Maxwell Laboratories, later Maxwell Technologies took over this development. Maxwell adopted the term Ultracapacitor from PRI and called them "Boost Caps"[5]to underline their use for power applications.Since the energy content of a capacitor increases with the square of the voltage, researchers were looking for a way to increase the breakdown voltage. Using an anode of a 200V high voltage tantalum electrolytic capacitor in 1994 David A. Evans developed an "Electrolytic-Hybrid Electrochemical Capacitor".[15][16]These capacitors combine features of electrolytic and electrochemical capacitors. They combine the high dielectric strength of an anode from an electrolytic capacitor, and the high capacitance with a pseudocapacitive metal oxide (ruthenium (IV) oxide) cathode from an electrochemical capacitor, yielding a hybrid. Evans' Capattery[17] had an energy content about a factor of 5 higher than a comparable tantalum electrolytic capacitor of the same size.[18]Their high costs limited them to specific military applications.Recent developments in lithium-ion capacitors are also hybrids. They were pioneered by FDK in 2007.[19]They combine an electrostatic double-layer electrode with a doped lithium-ion electrochemical battery electrode to generate high pseudocapacitance additional to high double-layer capacitance.Development of the double layer and pseudocapacitance model HelmholtzWhen a metal (or an electronic conductor) is brought in contact with a solid or liquid ionic-conductor (electrolyte), a common boundary (interface) among the two different phases emerges. Helmholtz[20]was the first to realize that charged electrodes immersed in electrolytic solutions repel the coions of the charge while attracting counterions to their surfaces. With the two layers of opposite polarity formed at the interface between electrode and electrolyte in 1853 he showed that an electrical double layer (DL) that is essentially a moleculear dielectric achieved electrostatic charge storage.[21] Below the electrolyte's decomposition voltage the stored charge is linearly dependent on the voltage applied.This early Helmholtz model predicted a constant differential capacitance independent from the charge density depending on the dielectric constant of the solvent and the thickness of the double-layer.[5][22][23] But this model, while a good foundation, does not consider important factors including diffusion/mixing of ions in solution, the possibility of adsorption onto the surface and the interaction between solvent dipole moments and the electrode.Simplyfied illustration of the potential development in the area and in the further course of a Helmholtz double layer.Gouy\Chapman [edit]Louis Georges Gouy in 1910 and David Leonard Chapman in 1913 both observed that capacitance was not a constant and that it depended on the applied potential and the ionic concentration. The ―Gouy-Chapman model‖ made significant improvements by introducing a diffuse model of the DL. In this model the charge distribution of ions as a function of distance from the metal surface allows Maxwell–Boltzmann statistics to be applied. Thus the electric potential decreases exponentially away from the surface of the fluid bulk.[5][24]Stern [edit]Gouy-Chapman fails for highly charged DLs. In order to resolve this problem Otto Stern in 1924 suggested the combination of the Helmholtz and Gouy-Chapman models. In Stern's model, some of the ions adhere to the electrode as suggested by Helmholtz, giving an internal Stern layer and some form a Gouy-Chapman diffuse layer.[25]The Stern layer accounted for ions' finite size and consequently ions have a closest approach to the electrode on the order of the ionic radius. The Stern model too had limitations, effectively modeling ions as point charges, assuming all significant interactions in the diffuse layer are Coulombic, assuming dielectric permittivity to beconstant throughout the double layer, and that fluid viscosity is constant above the slipping plane.[26]Grahame [edit]Thus, D. C. Grahame modified Stern in 1947.[27]He proposed that some ionic or uncharged species can penetrate the Stern layer, although the closest approach to the electrode is normally occupied by solvent molecules. This could occur if ions lost their solvation shell when the ion approached the electrode. Ions in direct contact with the electrode were called ―specifically adsorbed ions‖. This model proposed the existence of three regions. The inner Helmholtz plane (IHP) plane passing through the centres of the specifically adsorbed ions. The outer Helmholtz plane (OHP) passes through the centres of solvated ions at their distance of closest approach to the electrode. Finally the diffuse layer is the region beyond the OHP.Schematic representation of a double layer on an electrode (BMD) model. 1. Inner Helmholtz plane, (IHP), 2. Outer Helmholtz plane (OHP), 3. Diffuse layer, 4. Solvated ions (cations) 5. Specifically adsorbed ions (redox ion, which contributes to the pseudocapacitance), 6. Molecules of the electrolyte solventBockris/Devanthan/Müller [edit]In 1963 J. O'M. Bockris, M. A. V Devanthan, and K. Alex Müller[28]proposed a model (BDM model) of the double-layer that included the action of the solvent in the interface. They suggested that the attached molecules of the solvent, such as water, would have a fixed alignment to the electrode surface. This first layer of solvent molecules display a strong orientation to the electric field depending on the charge. This orientation has great influence on the permittivity of the solvent which varies with the field strength. The inner Helmholtz plane (IHP) passes through the centers of these molecules. Specifically adsorbed, partially solvated ions appear in this layer. The solvated ions of the electrolyte are outside the IHP. Through the centers of theseions pass a second plane, the outer Helmholtz plane (OHP). The region beyond the OHP is called the diffuse layer. The BDM model now is most commonly used. Trasatti/Buzzanca [edit]Further research with double layers on ruthenium dioxide films in 1971 by Sergio Trasatti and Giovanni Buzzanca demonstrated that the electrochemical behavior of these electrodes at low voltages with specific adsorbed ions was like that of capacitors. The specific adsorption of the ions in this region of potential could also involve a partial charge transfer between the ion and the electrode. It was the first step towards pseudo-capacitors.[22]Ph.D., Brian Evans Conway within the John Bockris Group At Imperical College, London 1947Conway [edit]Between 1975 and 1980 Brian Evans Conway conducted extensive fundamental and development work on the ruthenium oxide type of electrochemical capacitor. In 1991 he described the transition from ‗Supercapacitor‘ to ‗Battery‘ behavior in electrochemical energy storage and in 1999 he coined the term supercapacitor as explanation for increased capacitance by surface redox reactions with faradaic charge transfer between electrodes and ions.[1][29][30]His "supercapacitor" stored electrical charge partially in the Helmholtz double-layer and partially was the result of faradaic reactions with ―pseudocapacitance‖ charge transfer of electron and protons between electrode and electrolyte. The working mechanisms of pseudocapacitors are electrosorption, redox reactions and intercalation.Marcus[edit source | edit]The physical and mathematical basics of electron charge transfer without making chemical bonds leading to pseudocapacitance was developed by Rudolph A. Marcus. Marcus Theory is a theory to explain the rates of electron transfer reactions – the rateat which an electron can move or jump from one chemical species to another. It was originally formulated to address outer sphere electron transfer reactions, in which the two chemical species only change in their charge with an electron jumping. For redox reactions without making or breaking bonds Marcus theory takes the place of Henry Eyring's transition state theory which has been derived for reactions with structural changes. R.A. Marcus received the Nobel Prize in Chemistry in 1992 for this theory Storage principlesElectrostatic vs electrochemical energy storageCharge storage principles of different capacitor types and their inherent voltage progressionThe voltage behavior of supercapacitors and batteries during charging/discharging differs clearlyIn conventional capacitors such as ceramic capacitors and film capacitors the electric energy is stored in a static electric field permeates the dielectric between two metallic conducting plates, the electrodes. The electric field originates by the separation ofcharge carriers. This charge separation creates a potential between the two electrodes, which can be tapped via an external circuit. The total energy stored in this arrangement increases with the amount of stored charge and the potential between the plates. The amount of charge stored per unit voltage is essentially a function of the size, the reciprocal value of the distance, and the material properties of the dielectric, while the potential between the plates is limited by the dielectric's breakdown field strength. The dielectric controls the capacitor's voltage.Conventional capacitors are also called electrostatic capacitors. The potential of a charged capacitor decreases linearly between the electrodes. This static storage also applies for electrolytic capacitors in which most of the potential decreases over the thin oxide layer of the anode. The electrolyte as cathode may be a little bit resistive so that for ―wet‖ electrolytic capacitors a small amount of the potential decreases over the electrolyte. For electrolytic capacitors with high conductive solid polymer electrolyte this voltage drop is negligible.Electrochemical capacitors do not have a conventional solid dielectric that separates the charge. The capacitance value of an electrochemical capacitor is determined by electrostatic and electrochemical principles:Electrostatic storage of the electrical energy is achieved by charge separation in a Helmholtz double layer at the interface between the surface of a conductor electrode and an electrolytic solution electrolyte. This capacitance is called double-layer capacitance.Electrochemical storage of the electrical energy is achieved by redox reactions with: specifically adsorbed ions from the electrolyte; intercalation of atoms in the layer lattice(晶格层); or underpotential deposition of hydrogen or metal adatoms in surface lattice sites that results in a reversible faradaic charge-transfer on the electrode. This capacitance is called pseudocapacitance and is faradaic in origin.[5]Double-layer capacitance and pseudocapacitance combine to provide a supercapacitor's capacitance value.[2][3]Because each supercapacitor has two electrodes, the potential of the capacitor decreases symmetrically over both Helmholtz layers, whereby a little voltage drop across the ESR of the electrolyte achieved.Both the electrostatic and the electrochemical storage are linear with respect to the total charge. This linear behavior implies that the voltage across the capacitor is linear with respect to the amount of stored energy. This linear voltage gradient differs from electrochemical batteries, in which the voltage across the terminals remains independent of the charged energy, providing a constant voltage.Electrostatic double-layer capacitanceSimplified view of a double-layer of negative ions in the electrode and solvated positive ions in the liquid electrolyte, detached from each other through a layer of polarized molecules of the solvent.An electrical double layer is generated by applying a voltage to an arrangement of an electrode and an electrolyte. According to the voltage polarity, the dissolved and solvated ions in the electrolyte move to the electrodes. Two layers of ions are generated. One is in the surface of the electrode. The other, with opposite polarity, is the dissolved ions in the adjacent liquid electrolyte. These layers of opposite ions are separated by a monolayer of isolating molecules of the solvent, such as water. The layers of isolating molecule, the inner Helmholtz plane (IHP), adhere by physical adsorption on the surface of the electrode and separate the opposite ions from each other, building a molecular dielectric(电介质). The amount of charge in the electrode is matched by the same magnitude of counter-charges in the outer Helmholtz plane (OHP). These phenomena can be used to store electrical charges. The stored charge in the IHP forms an electric field that corresponds to the strength of the applied voltage. It is only effective in the molecular layer of the solvent molecules and is static in origin.The "thickness" of a charged layer in the metallic electrode, i.e., the average extension perpendicular to the surface, is about 0.1 nm. It mainly depends on the electron density because the atoms in solid electrodes are stationary. In the electrolyte, the thickness depends on the size of the molecules of the solvent and of the movement and concentration of ions in the solvent. It ranges from 0.1 to 10 nm, and is described by the Debye length. The sum of the thicknesses is the total thickness of a double layer.Field strength [edit]The small thickness of the inner Helmholtz plane creates a strong electric field E. At a potential difference of, for example, U = 2V and a molecular thickness of d = 0.4 nm, the electric field strength will beThe voltage proof of aluminum oxide, the dielectric layer of aluminum electrolytic capacitors is approximately 1.4 nm/V. For a 6.3 V capacitor therefore the layer is 8.8 nm. The electric field is 6.3 V/8.8 nm = 716 kV/mm.The double-layer's field strength of about 5000 kV/mm is unrealizable in conventional capacitors with conventional dielectrics. No dielectric material could prevent charge carrier breakthrough. In a double-layer capacitor the chemical stability of the molecular bonds of the solvent molecules prevents breakthrough.[31]The forces that cause the adhesion are physical, not chemical, forces. Chemical bonds exist within the adsorbed molecules, but they are polarized. The magnitude of the electrical charge that can accumulate in the layers corresponds to the concentration of the adsorbed ions. Up to the electrolyte's decomposition voltage, this arrangement behaves like a capacitor in which the stored electrical charge is linearly dependent on the voltage applied.Structure and function of an ideal double-layer capacitor. Applying a voltage to the capacitor at both electrodes a Helmholtz double-layer will be formed separating the adhered ions in the electrolyte in a mirror charge distribution of opposite polarity. The double-layer is like the dielectric layer in a conventional capacitor, but with the thickness of a single molecule. The early Helmholtz model predicts a constant differential capacitance Cd independent from the charge density, depending on the dielectric constant ε and the charge layer separation δ.If the solvent of the electrolyte is water then with the influence of the high field strength, the permittivity ε is 6 (instead of 80 in normal conditions) and the layerseparation δ ca. 0.3 nm the value of differential capacitance predicted by the Helmholtz model is about 18 F/cm2.[22]This value can be used to calculate capacitance using the standard formula for conventional plate capacitors if only the surface of the electrodes is known. This capacitance can be calculated with:.The capacitance C is therefore greatest in devices made from materials with a high permit tivity ε, large electrode plate surface areas A and a small distance d between plates. The activated carbon electrodes have a surface area in the range of 10 to 40 µF/cm2. The double-layer distance is on the order of a few Angstroms (0.3-0.8 nm). This gives supercapacitors the highest capacitance values among the capacitors.[2][5]Because an electrochemical capacitor is composed of two electrodes the charge distribution in the Helmholtz layer at one electrode can be found in opposite polarity in the Helmholtz layer at the second electrode. The total capacitance value of is that of two capacitors connected in series. Because both capacitances have approximately the same value, the total capacitance is roughly half the capacitance of one electrode.Electrochemical pseudocapacitanceSimplified view of a double-layer with specifically adsorbed ions which have submitted their charge to the electrode to explain the faradaic charge-transfer of the pseudocapacitance.In a Helmholtz double-layer not only a static double-layer capacitance originates. Specifically adsorbed ions with redox reactions, electrosorption and intercalation results in faradaic charge-transfer between electrolyte and surface of an electrodecalled pseudocapacitance. Double-layer capacitance and pseudocapacitance both contribute to the total capacitance value of a electrochemical capacitor.[2][3]The distribution of the amounts of both capacitances depends on the surface area, material and structure the of the electrodes.Redox reactions in batteries with faradaic charge-transfer between an electrolyte and a surface of an electrode are well known since decades. But these chemical processes are associated with chemical reactions of the electrode materials usually with attendant phase changes. Although these chemical processes are relatively reversible, the charge and discharge of batteries often results in irreversibility reaction products of the chemical electrode-reagents. Accordingly, the cycle-life of rechargeable batteries is usually limited, and varies with the battery type. Additional the chemical processes are relatively slow extending the charge and discharge time of the batteries.An essential fundamental difference from redox reactions in batteries arises in supercapacitors, were a fast sequence of reversible redox processes with a linear function of degree of faradaic charge transfers take place. This behavior is the basic function of a new class of capacitance, the pseudocapacitance. Pseudocapacitance comprise fast and reversible faradaic processes with charge transfer between electrolyte and the electrode and is accomplished through reduction-oxidation reactions (redox reactions), electrosorption and intercalation processes in combination with the nonfaradaic formation of an electric double-layer. Capacitors with a high amount of pseudocapacitance are called pseudocapacitors.Applying a voltage at the capacitor terminals the polarized ions or charged atoms in the electrolyte are moving to the opposite polarized electrode forms a double-layer. Depending on the structure or the surface material of the electrode a pseudocapacitance can originate when specifically adsorbed cations pervades(遍及) the double-layer proceeding in several one-electron stages an excess of electrons. The electrons involved in the faradaic processes are transferred to or from valence-electron states (orbitals) of the redox electrode reagent. The electrons enter the negative electrode and flow through the external circuit to the positive electrode were a second double-layer with an equal number of anions has formed. But these anions will not take the electrons back. They are present on the surface of the electrode in the charged state, and the electrons remain in the quite strongly ionized and "electron hungry" transition-metal ions of the electrode. This kind of pseudocapacitance has a linear function within narrow limits and is determined by the potential-dependent degree of coverage of surface with the adsorbed anions from the electrolyte. The storage capacity of the pseudocapacitance with an electrochemical charge transfer takes place to an extent limited by a finite quantity of reagent or of available surface.Discharging the pseudocapacitance the reaction of charge transfer is reversed and the ions or atoms leave the double-layer and move into the electrolyte distributing randomly in the space between both electrodes.Unlike in batteries in pseudocapacitors the redox reactions or intercalation processes with faradaic charge-transfer do not result in slow chemical processes with chemical reactions or phase changes of the electrode materials between charge and discharge. The atoms or ions contribute to the pseudocapacitance simply cling[32]to the atomic structure of the electrode and charges are distributed on surfaces by physical adsorption processes that do not involve the making or breaking of chemical bonds. These faradaic charge transfer processes for charge storing or discharging employed in pseudocapacitors are very fast, much faster than the chemical processes in batteries.Confinement of solvated ions in pores, such as those present in carbide-derived carbon (CDC). As the pore size approaches the size of the solvation shell, the solvent molecules are removed, resulting in larger ionic packing density and increased charge storage capability.The ability of electrodes, to accomplish pseudocapacitance effects like redox reactions of electroactive species, electrosorption of H or metal ad-atoms or intercalation, which leads to a pseudocapacitance, strongly depend on the chemical affinity of electrode materials to the ions sorbed on the electrode surface as well as on the structure and dimension of the electrode pores. Materials exhibiting redox behavior for use as electrodes in pseudocapacitors are transition-metal oxides inserted by doping in the conductive electrode material like active carbon as well as conducting polymers such as polyaniline or derivatives of polythiophene covering the surface of conductive electrode material.Pseudocapacitance may also originates by the structure and especially by the pore size of the electrodes. The use of carbide-derived carbons(CDCs) or carbon nanotubes /CNTs for electrodes provides a network of very small pores formed by nanotube entanglement. These carbon nanoporous with diameters in the range of <2 nm can be referred to as intercalated pores. Solvated ions in the electrolyte can‘t enter these small pores but de-solvated ions which have reduced their ion dimensions are able to enter resulting in larger ionic packing density and increase charge storage capability. The tailored sizes of pores in nano-structured carbon electrodes can maximize ion confinement, increasing specific capacitance by faradaic H2adsorption treatment(?). Occupation of these pores by de-solvated ions from the electrolyte。
物理实验报告英文版7

iv
Table of Contents
Title Page Authorization Page Signature Page Acknowledgements Table of Contents List of Figures List of Tables Abstract Chapter1 Introduction 1.1 Structure of Carbon Nanotubes . . . . . . . . . . . . . . . . . . . . 1.2 Electronic properties of Carbon Nanotubes . . . . . . . . . . . . . . Chapter2 Superconductivity in 0.4nm Carbon Nanotubes array 2.1 The band structure of 0.4nm Carbon Nanotubes . . . . . . . . . . 2.2 Meissner effect in 0.4nm Carbon Nanotubes array . . . . . . . . . 2.3 The model of coupled one-dimensional superconducting wires . . . 2.4 Motivation and scope of the thesis . . . . . . . . . . . . . . . . . . i ii iii iv v vii xi xii 1 3 4 8 9 9 12 13
July 2008, Hong Kong
HKUST Library Reproduction is prohibited without the author’s prior written consent
Superconductivity in metal rich Li-Pd-B ternary Boride

Superconductivity in Metal Rich Li-Pd-B Ternary Boride K. Togano1, P. Badica1, Y. Nakamori1, S. Orimo1, H. Takeya2 and K. Hirata21Institute for Materials Research, Tohoku University, 2-1-1, Katahira,Aoba-ku, Sendai 980-8577, Japan2National Institute for Materials Science, 1-2-1, Sengen, Tsukuba 305-0047, Japan8K superconductivity was observed in the metal rich Li-Pd-B ternary system. Structural, microstructural, electrical and magnetic investigations for various compositions proved that Li2Pd3B compound, which has a cubic structure composed of distorted Pd6B octahedrons, is responsible for the superconductivity. This is the first observation of superconductivity in metal rich ternary borides containing alkaline metal and Pd as a late transition metal. The compound prepared by arc melting has high density, is stable in the air and has an upper critical field, H c2(0), of 6T.PACS numbers: 74.70.Dd, 74.25.Ha, 74.25.Fy, 74.25.OpThe search for superconductivity in boride compounds initiated in 1949 with the discovery of TaB (T c=4K) [1]. However, most works were done in the 1970’s and 1980’s resulting in the discovery of many binary and ternary superconducting borides, almost all of which involve either transition metal elements, rare-earth elements and platinum group elements of Ru, Rh, Os, Ir and Pt as metal constituents [2]. Despite of these efforts, the transition temperature T c of binary and ternary borides remained below 12K and could not exceed 23K, which was the highest T c of intermetallic compounds recorded by A15 Nb3Ge in 1973 [3]. It is interesting that no Pd binary or ternary boride was reported in stable condition, although Pd belongs to platinum group and gives the highest T c in the case of quaternary borocarbide system (RE)(TM)2B2C, where RE=rare earth metal and TM=Ni,Pd or Pt [4].Recent discovery of 39K superconductivity in MgB2 [5] has led to resurgence of interest in boride compounds as possible high temperature superconductor. It is surprising that such high T c was attained for simple binary boride with light alkaline earth element, Mg, as a metallic constituent. Since then, there have been several experimental and theoretical studies to search for high T c borides extending the metallic constituent to alkaline and alkaline earth elements. In these, of particular interest was the prediction of high temperature superconductivity in Li1-x BC system [6], however, to date, no superconductivity was reported for this system [7].In this paper, we report the discovery of superconductivity around 8K in a metal rich boride of Li2Pd3B compound with cubic structure. This is the first report for superconductivity in ternary borides containing alkaline metal and Pd as aplatinum group element and not containing any rare-earth and transition metal elements. The result is expected to provide a possible road for searching a new family of boride superconductors with high transition temperature.The samples were prepared by arc-meltingprocess in order to attain high-density material that is adequate to measure electrical properties. In order to minimize the loss of Li by evaporation, we employed a two-step arc melting process. Initially, the Pd-B binary alloy buttons were prepared by conventional arc melting method from the mixture of appropriate amounts of Pd (99.9%) and B (99.5 %). We prepared four alloys with different Pd:B ratios of 3:2, 5:2, 3:1 and 5:1. By this alloying, the melting point of the materials can be lowered below the boiling point of Li at 1atm. Weight loss during the first arc melting step, was negligible. The alloying of Li was done in the second arc melting processing step. A small block of Pd-B alloy (~200mg) obtained by crushing the button was placed on a small piece of Li plate (10-50mg) freshly cut from the Li ingot (>99%). The melting was done in ~1atm argon atmosphere and the arc current was controlled to the necessary minimum; once the Pd-B alloy melted, the reaction with Li occurred and developed very fast probably due to the self-heating generated in the exothermic-type reaction. The loss of Li was inevitable making difficult the control of Li concentration in the final products. Therefore, the Li concentration in the obtained Li-Pd-B alloy was estimated from the weight gain of the Pd-B alloy that is considered to have a constant weight during arc-synthesis.Temperature dependence of magnetization-1.5-1.0-0.53456789101112Temperature (K)M(emu/g)was measured for the samples at 100Oe by a superconducting quantum interference device (SQUID) magnetometer. A sharp drop of magnetization at around 7-8K, which is the characteristic signature of superconductivity, was observed for a variety of compositions examined in this work. The largest diamagnetic signal (Fig. 1) was obtained for the sample with an estimated composition of approximately Li2Pd3B. The onset of the transition is 8K as shown in the inset of Fig. 1. The zero-field-cooling (ZFC) curve shows an almost full shielding effect, while the field-cooling (FC) curve shows a low Meissner effect of the order of 1% due to flux trapping. The sample has uniform solidification microstructure (Fig. 2), composed of grains with a few hundreds µ m size with cellular dendrites inside the grain. Many cracks were observed along the grain boundaries and sub-grain boundaries. The powder X-ray diffraction pattern for this sample is Figure 1 Magnetization vs. temperature curves for the Li2Pd3B sample measured in zero-field-cooling (ZFC) and field-cooling (FC) arrangements in the magnetic field of 100Oe. Inset is showing the FC curve in detail.040008000120001020304050607080905305224405326104425315215203335104304223324214203314114104003213202223113102212202112101111102θ (°)I n t e n s i t y (a .u .)shown in Fig. 3. All of the peaks can be ascribed to Li 2Pd 3B compound, which was recently reported by Eibenstein and Jung [8]. The crystal structure is cubic and composed of distorted Pd 6B octahedrons. No apparent peak of impurity phase was observed. The samples with estimated compositions different from Li 2Pd 3B showed a broader transition with smaller diamagnetic signal, and at the same time extra peaks belonging to unidentified phases occurred in the XRD patterns. From these results, we conclude that the cubic Li 2Pd 3B compound is responsible for the observed 8K- superconductivity. The sample is stable in the00.000250.000500.00075123456789101112Temperature (K)R e s i s t a n c e (Ω)air and showed no significant difference of diamagnetic signal after 1 week.Figure 2 Optical microstructure on the crosssection of the arc melted Li 2Pd 3B button.Figure 4 Temperature-dependent resistivity inapplied magnetic fields of 0, 0.05, 0.1, 0.2, 0.5,1, 2, 3, 4, 5T for the Li 2Pd 3B bulk sample (a few mm size). Applied current was 1mA for a 4-probe configuration.Figure 4 presents the temperature-dependent resistivity in applied magnetic fields up to 5T for Li 2Pd 3B sample. The sample was a bulk (a few mm size) obtained by crushing the final arc melted product (button). Measuring applied current was 1mA. Under zero field, the curve drops sharply with the onset temperature of 8.2 K and the transition width of 0.6K. The onset temperature is slightly higher than that obtained from the magnetization measurement. In applied magnetic field, the curve shows the parallel shift to lower temperature, which is characteristic for the metallic superconductors. In Figure 5 is given the onset temperature as a function of magnetic field. The curve shows a positive curvature near to T c , similar to the polycrystalline borocabides [9] and MgB 2 [10]. Except this region, the curve is linear, whose gradient dH c2/dT is -0.84T/K. LinearFigure 3 XRD powder pattern of the Li 2Pd 3Bsample synthesized by arc melting.2.55.07.5Temperature (K)H c 2 (T )extrapolation of the curve gives the upper critical field H c2(0)of 6.25T. In summary, we found that the cubic Li 2Pd 3B compound is a superconductor with critical temperature of about 8K and upper critical field H c2(0) of 6T. The compound prepared by arc-melting has a uniform structure and high density, and is stable in the air. This is the first observation of superconductivity in ternary metal rich borides composed of alkaline metal, platinum group element and boron. The result is expected to provide a new direction for searching new types of boride superconductors with high superconducting transition temperature.The authors would like to thank Mr. T. Kondo and Mr. T. Kudo for technical assistance during experiments. The study was carried out as a part of “Ground-based Research Announcement for Space Utilization” promoted by Japan Space Forum.References[1] R. Kiessling, Acta Chem. Scand. 3, 603 (1949). [2] C. Buzea and T. Yamashita, Supercond. Sci.Technol. 14, R115 (2001).[3] J.R. Gavaler, Appl. Phys. Lett. 23, 480 (1973). [4] J.R. Cava, H. Takagi, B. Batlogg, H.W.Zandbergen, J.J. Krajewski, W.P. Peck, Jr., R.B. van Dover, R.J. Felder, T. Siegrist, K. Muzuhashi, J.O. Lee, H. Eisaki, S.A. Carter and S. Uchida, Nature 367, 146 (1994).Figure 5 H c2(T) plot defined by the onsettemperature of resistive transition measured in magnetic fields for the Li 2Pd 3B sample.[5] J. Nagamatsu, N. Nakagawa, T. Muranaka, Y .Zenitani and J. Akimitsu, Nature 410, 63 (2001). [6] H. Rosner, A. Kitaigorodsky and W.E. Picket,Phys. Rev. Lett. 88, 127001 (2002).[7] L. Zhao, P. Klavins and Kai Liu, J. Appl. Phys. 93,8653 (2003).[8] U. Eibenstein and W. Jung, J. Solid State Chem.133, 21 (1997).[9] H. Takagi, R.J. Cava, H. Eisaki, J.O. Lee, K.Mizuhashi, B. Batlogg, S. Uchida, J.J. Krajewski and W.F. Peck, Jr., Physica C228, 389 (1994). [10] Y . Takano, H. Takeya, H. Fujii, H. Kumakura, T.Hatano, K. Togano, H. Kito and H. Ihara, Appl. Phys. Lett. 78, 2914 (2001).。
关于拓扑超导的英文演讲

关于拓扑超导的英文演讲Topological superconductivity is a fascinating topic in the field of condensed matter physics that has garnered significant attention in recent years. In this speech, I will provide an overview of the concept, its potential applications, and the ongoing research in this exciting field.Firstly, let's understand what topological superconductivity is. Superconductivity is a quantum phenomenon that occurs at very low temperatures, where certain materials can conduct electricity without any resistance. This property is due to the formation of Cooper pairs, which are pairs of electrons with opposite spins. Topological superconductivity refers to a special class of superconductors where the Cooper pairs exhibit an additional quantum property known as non-Abelian statistics.Non-Abelian statistics means that the quantum wavefunction of the system is not invariant under the exchange of particles. This unique characteristic holds the potential for storing and manipulating quantum information, making topological superconductors a promising platform for developing quantum computers. Unlike conventional superconductors, which are described by Abelian statistics, the non-Abelian nature of topological superconductivity provides protection against certain types of local perturbations and disturbances, making them more stable against noise.The study of topological superconductivity is closely connected to the field of topological insulators. Topological insulators are materials that have a unique electronic band structure that results in conducting surface states while remaining insulating in the bulk. This distinct behavior arises due to the nontrivial topology of the electron wavefunctions. By introducing superconductivity into topological insulators, researchers have been able to realize topological superconductivity.One of the most exciting prospects of topological superconductivity is its potential for hosting Majorana fermions. Majorana fermions are hypothesized particles that are their own antiparticles, meaning they can annihilate and reappear as their own particle. Majorana fermions have distinct properties that make them attractive for quantumcomputing, as they are expected to have a higher resistance to decoherence. Decoherence is a phenomenon that can disrupt quantum states and is a major challenge in quantum computing.Numerous experimental efforts have been dedicated to the search for evidence of Majorana fermions in topological superconductors. One of the most notable experiments is the creation of a hybrid structure called a topological superconductor nanowire. This nanowire, made of materials with strong spin-orbit coupling and proximity-induced superconductivity, exhibits the predicted signatures of Majorana fermions. These experimental advancements have sparked great excitement and sparked further research in the field of topological superconductivity.Apart from quantum computing, topological superconductivity also has potential applications in other areas, such as topological quantum computation and fault-tolerant quantum memories. Researchers are actively exploring the possibilities of using the unique properties of topological superconductors to create new technologies that can revolutionize various fields.In conclusion, topological superconductivity is a captivating area of research with great potential for quantum technologies. Its non-Abelian nature and the possible existence of Majorana fermions make it a promising platform for quantum computing and other applications. Continued experimental efforts and theoretical investigations are crucial in unraveling the mysteries and realizing the full potential of topological superconductivity. The future of this field holds exciting possibilities that could shape the future of quantum technology.。
超导现象-英汉双语

Superconductivity超导现象One of the earliest properties investigated in the laboratories at Leiden was the resistance of metal wires. It was measured by finding the voltage, or potential difference, between the ends of a wire when a known current was flowing through it. Whenever the current is doubled, the voltage is also doubled according to Ohm's law, and the resistance is voltage over current (R=v/c). With some metals such as copper, iron and platinum, the resistance dropped smoothly down with falling temperature, until at 40°K it was only perhaps a hundredth of its value at 0℃. With others, notably lead, mercury and tin, there was a temperature, different for each one but well below 20°K, at which the resistance dropped to nothing at all. A hundredth of a degree above this critical temperature the resistance was normal, like those of copper, iron and platinum; but a hundredth of a degree below, it was zero or too small to measure.参考译文:金属丝的电阻是莱顿实验室最早研究的金属特性之一。
Magnetic Ordering and Superconductivity in the RE$_2$Ir$_3$Ge$_5$ (RE = Y, La-Tm, Lu) Syste

Abstract
We report structure, electrical resistivity, magnetic susceptibility, isothermal magnetization and heat-capacity studies on polycrystalline samples of the intermetallic series RE2Ir3Ge5 (RE = Y, La, Ce-Nd, Gd-Tm, Lu) from 1.5 to 300 K. We find that the compounds for RE = Y, La-Dy, crystallize in the tetragonal Ibam (U2Co3Si5 type) structure whereas the compounds for RE= Er-Lu, crystallize in a new orthorhombic structure with a space group Pmmn. Samples of Ho2Ir3Ge5 were always found to be multiphase. The compounds for RE = Y to Dy which adopt the Ibam type structure show a metallic resistivity whereas the compounds with RE = Er, Tm and Lu show an anomalous behavior in the resistivity with a semiconducting increase in ρ as we go down in temperature from 300 K. Interestingly we had earlier found a positive temperature coefficient of resistivity for the Yb sample in the same temperature range. We will compare this behavior with similar observations in the compounds RE3Ru4Ge13 and REBiPt. La2Ir3Ge5 and Y2Ir3Ge5 show bulk superconductivity below 1.8 K and 2.5 K respectively. Our results confirm that Ce2Ir3Ge5 shows a Kondo lattice behavior and undergoes antiferromagnetic ordering below 8.5 K. Most of the other compounds containing magnetic rare-earth elements undergo a single antiferromagnetic transition at low temperatures (T≤12 K) while Gd2Ir3Ge5, Dy2Ir3Ge5 and Nd2Ir3Ge5 show multiple transitions. The TN ’s for most of the compounds
丹佛斯 EKC 315A 超充控制器用户手册说明书
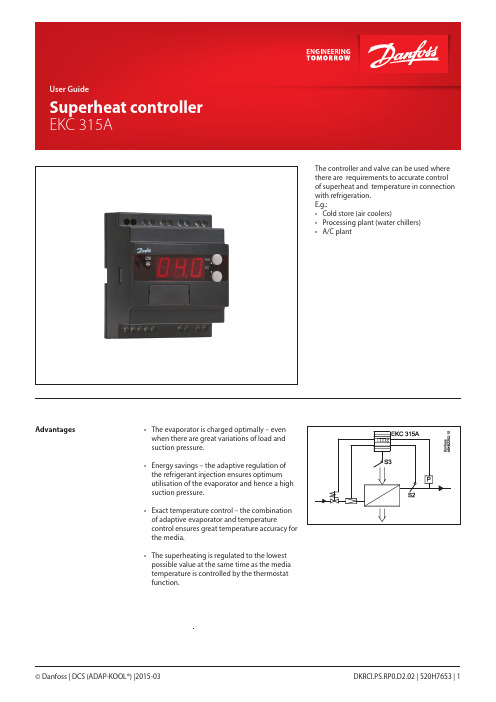
DKRCI.PS.RP0.D2.02 | 520H7653 | 1© Danfoss | DCS (ADAP-KOOL®) |2015-03Advantages• The evaporator is charged optimally – even when there are great variations of load and suction pressure.• Energy savings – the adaptive regulation of the refrigerant injection ensures optimum utilisation of the evaporator and hence a high suction pressure.• Exact temperature control – the combination of adaptive evaporator and temperaturecontrol ensures great temperature accuracy for the media.• The superheating is regulated to the lowest possible value at the same time as the media temperature is controlled by the thermostat function.The controller and valve can be used where there are requirements to accurate control of superheat and temperature in connection with refrigeration.E.g.:• Cold store (air coolers)• Processing plant (water chillers)•A/C plantUser Guide | Superheat controller, EKC 315A© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653 | 2IntroductionFunctions• Regulation of superheat • Temperature control • MOP function• ON/OFF input for start/stop of regulation• Input signal that can displace the superheat reference or the temperature reference• Alarm if the set alarm limits are exceeded • Relay output for solenoid valve • PID regulation• Output signal following the temperature showing in the display SystemThe superheat in the evaporator is controlled by one pressure transmitter P and one temperature sensor S2.The valve can be one of the following types:• ICM• AKV (AKVA)ICM is an electronically, directly run engine valve, controlled by an ICAD type actuator. It is used with a solenoid valve in the liquid line.TQ valveThe controller can also control a TQ type valve. This valve has been discontinued from the product range, but the settings are still described in this manual.AKV is a pulsating valve.Where the AKV valve is used it also functions as solenoid valve.Temperature control is performed based on a signal from tem-perature sensor S3 which is placed in the air current before the evaporator. Temperature control is in the shape of an ON/OFFthermostat that shuts off the liquid flow in the liquid line.User Guide | Superheat controller, EKC 315A© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653| 3OperationSuperheat functionYou may choose between two kinds of superheat, either:• Adaptive superheat or • Load-defined superheatMOPThe MOP function limits the valve’s opening degree as long as the evaporating pressure is higher than the set MOP value.Override functionVia the analog input a displacement can be made of the tempera-ture reference or of the superheat reference. The signal can either be a 0-20 mA signal or a 4-20 mA signal. The reference can be displaced in positive or negative direction.External start/stop of regulationThe controller can be started and stopped externally via a contact function connected to input terminals 1 and 2. Regulation is stopped when the connection is interrupted. The function must be used when the compressor is stopped. The controller then closes the solenoid valve so that the evaporator is not charged with refrigerant.RelaysThe relay for the solenoid valve will operate when refrigeration is required. The relay for the alarm function works in such a way that the contact is cut-in in alarm situations and when the controller is de-energised.Modulating/pulsating expansion valveIn 1:1 systems (one evaporator, one compressor and one condens-er) with small refrigerant charge ICM is recommended.In a system with an AKV valve the capacity can be distributed by up to three valves if slave modules are mounted. The controller will displace the opening time of the AKV valves, so that they will not pulsate at the same time.Used as slave module is a controller of the type EKC 347.Analog outputThe controller is provided with an analog current output which can be set to either 0-20 mA or 4-20 mA. The signal will either fol-low the superheat, opening degree of the valve or the air tem-perature.When an ICM valve is in use, the signal is used for control of the valve via the ICAD actuator.PC operationThe controller can be provided with data communication so that it can be connected to other products in the range of ADAP-KOOL® refrigeration controls. In this way operation, monitoring and data collection can be performed from one PC – either on the spot or ina service company.User Guide | Superheat controller, EKC 315ASurvey of functions© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653 | 4User Guide | Superheat controller, EKC 315A © Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653| 5User Guide | Superheat controller, EKC 315A © Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653 | 6User Guide | Superheat controller, EKC 315A© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653| 7User Guide | Superheat controller, EKC 315A© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653 | 8There are LED’s on the front panel which will light up when the belonging relay is activated.The upper LED will indicate the valve’s opening degree. A short pulse indicates a small liquid flow and a long pulse a heavy liquid flow. The other LED will indicate when the controller calls for refrigeration.The three lowermost LED’s will flash, if there is an error in the regu-lation.In this situation you can upload the error code on the display and cancel the alarm by giving the uppermost button a brief push.DisplayThe values will be shown with three digits, and with a setting you OperationMenu surveyThe buttonsWhen you want to change a setting, the two buttons will give you a higher or lower value depending on the button you are push-ing. But before you change the value, you must have access to the menu. You obtain this by pushing the upper button for a couple of seconds - you will then enter the column with parameter codes. Find the parameter code you want to change and push the two buttons simultaneously. When you have changed the value, save the new value by once more pushing the two buttons simultane-ously. Gives access to the menu (or cutout an alarm) Gives access to changesSaves a changeExamples of operationsSet set-point1. Push the two buttons simultaneously2. Push one of the buttons and select the new value3. Push both buttons again to conclude the settingSet one of the other menus1. Push the upper button until a parameter is shown2. Push one of the buttons and find the parameter you want to change3. Push both buttons simultaneously until the parameter value is shown4. Push one of the buttons and select the new value5. Push both buttons again to conclude the settingSW =1.4xFunctionPara-meterMin.Max.FactorysettingNormal displayShows the actual superheat/ valve's opening degree/ temperature Define view in o17-KTemperature, superheating, or the temp. reference is displayed if the bottom button is pressed briefly.Define view in o17-%ReferenceSet the required set point --60°C 50°C 10Differentialr010.1 K 20 K 2.0Units (0=°C+bar /1=°F+psig)r05010External contribution to the reference r06-50 K50 K0Correction of signal from S2r09-50.0 K 50.0 K 0.0Correction of signal from S3r10-50.0 K 50.0 K 0.0Start / stop of refrigeration r12OFF On 0Define thermostat function(0= no thermostat function, 1=On/off thermostat)r141AlarmUpper deviation (above the temperature setting)A01 3.0 K 20 K 5.0Lower deviation (below the temperature setting)A02 1 K10 K3.0Alarm’s time delay A030 min.90 min.30Regulating parameters P: Amplification factor Kp n040.520 3.0I: Integration time Tn0530 s 600 s 120D: Differentiation time Td (0 = off)n060 s 90 s 0Max. value of superheat reference n09 2 K 50 K 6Min. value of superheat reference n10 1 K 12 K 4MOP (max = off)n110.0 bar 60 bar 60Period time (only when AKV/A valve is used)n13 3 s 10 s 6Stability factor for superheat control.Changes should only be made by trained staff n180105Damping of amplification around reference value Changes should only be made by trained staff n190.2 1.00.3Amplification factor for superheatChanges should only be made by trained staff n200.010.00.4Definition of superheat control 1=MSS, 2=LOADAPn21121Value of min. superheat reference for loads under 10%n221152Standby temperature when valve closed (TQ valve only)Changes should only be made by trained staff n260 K20 KStandby temperature when valve open (TQ valve only)Changes should only be made by trained staff n27-15 K 70 K 20Max. opening degreeChanges should only be made by trained staff n320100100Min. opening degreeChanges should only be made by trained staff n33100Miscellaneous Controller’s addresso03*0119-ON/OFF switch (service-pin message)o04*---Define valve and output signal:0: Off1: TQ. AO: 0-20 mA 2: TQ. AO: 4-20 mA 3: AKV, AO: 0-20 m 4: AKV, AO: 4-20 mA5: AKV, AO: EKC 347-SLAVE 6: ICM, AO: 0-20 mA / ICM OD%7: ICM, AO: 4-20 mA / ICM OD%o09070User Guide | Superheat controller, EKC 315A© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653| 9Factory settingIf you need to return to the factory-set values, it can be done in this way:- Cut out the supply voltage to the controller- Keep both buttons depressed at the same time as you recon n ect the supply voltageThe controller can give the following messages:E1Error message Fault in controllerE11Valve’s actuator temperature outside its range E15Cut-out S2 sensor E16Shortcircuited S2 sensor E17Cut-out S3 sensor E18Shortcircuited S3 sensorE19The input signal on terminals 18-19 is outside the range.E20The input signal on terminals 14-15 is outside the range (P0 signal)A1Alarm messageHigh-temperature alarm A2Low-temperature alarm A11No refrigerant has been selectedDefine input signal on the analog input AIA:0: no signal,1: Temperature setpoint. 0-20 mA 2: Temperature setpoint. 4-20 mA3: Displacement of superheat reference. 0-20 mA 4: Displacement of superheat reference. 4-20 mA o104Set supply voltage frequency o1250 Hz60 HzSelect display for ”normal picture”(Display the item indicated in parenthesis by briefly pressing the bottom button) 1: Superheat (Temperature)2: Valve’s opening degree (Superheat)3: Air temperature (Temperature reference)o17131Manual control of outputs:OFF: no manual control1: Relay for solenoid valve: select ON 2: AKV/A output: select ON3: Alarm relay activated (cut out)o18off3OffWorking range for pressure transmitter – min. valueo20-1 bar 60 bar -1.0Working range for pressure transmitter – max. valueo21-1 bar60 bar 12(Setting for the function o09, only AKV and TQ)Set the temperature value or opening degree where the output signal must be minimum (0 or 4 mA)o27-70°C 160°C -35(Setting for the function o09, only AKV and TQ)Set the temperature value or opening degree where the output signal must be maximum (20 mA)o28-70°C 160°C 15Refrigerant setting1=R12. 2=R22. 3=R134a. 4=R502. 5=R717. 6=R13. 7=R13b1. 8=R23. 9=R500. 10=R503. 11=R114.12=R142b. 13=User defined. 14=R32. 15=R227. 16=R401A.17=R507. 18=R402A. 19=R404A. 20=R407C. 21=R407A. 22=R407B. 23=R410A. 24=R170. 25=R290. 26=R600. 27=R600a. 28=R744. 29=R1270. 30=R417A. 31=R422A. 32=R413A. 33=R422D. 34=R427A. 35=R438Ao300350ServiceTQ valve's actuator temperatureu04°C Reference of the valve's actuator temperature u05°C Analog input AIA (18-19)u06mA Analog output AO (2-5)u08mA Read status of input DI u10on/off Thermostat cut-in time u18min.Temperature at S2 sensor u20°C Superheatu21K Superheat referenceu22K Read AKV valve’s opening degree u24%Read evaporating pressure u25bar Read evaporating temperature u26°C Temperature at S3 sensor u27°C Temperature referenceu28°C Read signal at pressure transmitter input u29mA*) This setting will only be possible if a data communication module has been installed in the controller.User Guide | Superheat controller, EKC 315A© Danfoss | DCS (ADAP-KOOL®) | 2015-03DKRCI.PS.RP0.D2.02 | 520H7653 | 10Installation considerationsAccidental damage, poor installation, or site conditions, can give rise to malfunctions of the control system, and ultimately lead to a plant breakdown.Every possible safeguard is incorporated into our products to prevent this. However, a wrong installation, for example, could still present problems. Electronic controls are no substitute for normal, good engineering practice.Danfoss wil not be responsible for any goods, or plant compo-nents, damaged as a result of the above defects. It is the installer's responsibility to check the installation thoroughly, and to fit the necessary safety devices.Particular attention is drawn to the need for a “force closing” signal to controllers in the event of compressor stoppage, and to the requirement for suction line accumulators.Your local Danfoss agent will be pleased to assist with further advice, etc.Appendix 1Interaction between internal and external start/stop functions and active functions.Appendix 2Cable length for the TQ actuatorThe actuator must be supplied with 24 V a.c. ± 10%.To avoid excessive voltage loss in the cable to the actuator, use a thicker cable for large distances.Wire cross sectionCable lengthInternal Start/stop Off Off On On External Start/stop (DI)Off On Off On Refrigeration (DO2)Off OnTQ actuatorStandbytemperatureRegulatingExpansion valve relay Off On Temperature monitoring No Yes Sensor monitoring Yes Yes ICM Closed RegulatingThe two types of regulation for superheat are, as follows:Adaptive superheatRegulation is here based on the evaporator’s load by means of MSS search (MSS = lowest permissible superheat).(The superheat reference is lowered to the exact point where instability sets in).The superheat is limited by the settings for min.and max.super-heat.Load-defined superheatThe reference follows a defined curve.This curve is defined by three values: the closing value, the min. value and the max. value. These three values must be selected in such a way that the curve is situated between the MSS curve and the curve for average temperature difference ∆Tm (temperature difference between media temperature and evaporating temperature.Setting example = 4, 6 and 10 K).Start of controllerWhen the electric wires have been connected to the controller, the following points have to be attended to before the regulation starts:1. Switch off the external ON/OFF switch that starts and stops the regulation.2. Follow the menu survey on page 8, and set the various para-meters to the required values.3. Switch on the external switch, and regulation will start.If the superheating fluctuatesWhen the refrigerating system has been made to work steadily, the controller’s factory-set control parameters should in most cases provide a stable and relatively fast regulating system.If the system however fluctuates this may be due to the fact that too low superheat parameters have been selected:If adaptive superheat has been selected:Adjust: n09, n10 and n18.If load-defined superheat has been selected:Adjust: n09, n10 and n22.Alternatively it may be due to the fact that the set regulation parameters are not optimal.If the time of oscillation is longer than the integration time:(Tp> Tn, (Tnis, say, 240 seconds))1. Increase Tnto 1.2 times Tp2. Wait until the system is in balance again3. If there is still oscillation, reduce Kpby, say, 20%4. Wait until the system is in balance5. If it continues to oscillate, repeat 3 and 4If the time of oscillation is shorter than the integration time:(Tp< Tn, (Tnis, say, 240 seconds))1. Reduce Kpby, say, 20% of the scale reading2. Wait until the system is in balance3. If it continues to oscillate, repeat 1 and 2.4. Follow the actual room temperature or superheat on the display.(On terminals 2 and 5 a current signal can be transmitted which represents the display view. Connect a data collection unit, if applicable, so that the temperature performance can be followed).If the superheat has excessive underswing during start-upIf you regulate with valve type ICM or AKV:Adjust n22 a little bit up and/or n04 a little bit down.If you regulate with valve type TQ:Adjust n26 a littlle bit downList of literatureInstructions RI8GT (extract from this manual).Here you can see how controllers are mounted and programmed.Installation guide for extended operation RC8ACHere you can see how a data communication connection to ADAP-KOOL® Refrigeration control systems can be estab-lished.Danfoss can accept no responsibility for possible errors in catalogues, brochures and other printed material. Danfoss reserves the right to alter its products without notice. This also applies to products already on order provided that such alternations can be made without subsequential changes being necessary in specifications already agreed.All trademarks in this material are property of the respecitve companies. Danfoss and Danfoss logotype are trademarks of Danfoss A/S. All rights reserved.。
凝聚态物理实验第三章第二节

Related readings:
“More is Different – One more time” in “More is different” edited by Ong and Bhatt “A different universe – reinventing physics from the bottom DOWN” by Laughlin
Reference: The Nobel Prize lecture by Phillip W. Anderson
“Disordered electronic systems”, Patrick A. Lee, Rev. Mod. Phys. 57, 287 (1985) “Localization Yesterday, Today and Tomorrow” by Ramakrishnan in “More is Different”
Born 1923
According to ’s analysis of the influence of scientific research papers, Anderson is the most creative physicist in the world, followed by Steve Weinberg and Ed Witten.
extended state with mean free path l
localized state with localization length
Question:
• So far it seems the only role of disorder is to cause scattering of the Bloch waves without modifying the behavior of electrons qualitatively. What if we put in lots of impurities with strongly different impurity potential? Can the disorders cause fundamental change of the electronic
freeze

freezeTitle: Freeze: The Intriguing Phenomenon and its ImpactsIntroduction:Freeze is a natural phenomenon that occurs when a substance experiences a drop in temperature to the point where it transitions from a liquid state to a solid state. This process is commonly associated with water, but it can happen to other substances as well. The freezing process is not only a fascinating scientific event but also plays a significant role in various aspects of our lives. In this document, we will delve into the remarkable world of freeze, exploring the science behind it while highlighting its effects on the environment, technology, and daily life.The Science Behind Freeze:Freezing is a phase transition that occurs when the temperature of a substance reaches its freezing point. At this point, the kinetic energy of the molecules within the substance decreases, causing them to lose their freedom of movement. The molecules become arranged in a regular,crystalline structure, resulting in a solid state. The freezing point of a substance is unique and depends on various factors such as pressure and impurities present in the substance.Water, being the most abundant liquid on Earth, freezes at 0 degrees Celsius (32 degrees Fahrenheit) under normal atmospheric conditions. Interestingly, the process of freezing water is accompanied by a decrease in volume, leading to the expansion of the substance. This expansion can have significant implications, such as the formation of ice in cracks in rocks, leading to their fragmentation over time.Environmental Impacts:Freezing plays a crucial role in Earth's climate system and has various environmental impacts. The freezing of water bodies, such as lakes and rivers, during winter has a profound effect on the ecosystem. The ice cover stabilizes water temperature, provides a habitat for certain organisms, and protects underwater life from extreme cold. Additionally, the seasonal freezing and melting of polar ice caps regulate global sea levels, impacting coastlines worldwide.Climate change has led to alterations in freezing patterns, causing significant concerns. The retreat of polar ice caps and the decrease in ice cover on lakes affect the delicate balance of ecosystems, disrupt animal migration routes, and impact local economies, such as tourism and fishing industries. Understanding the freezing process and its environmental consequences will help in predicting and mitigating the effects of climate change.Technological Applications:The study of freeze and its practical applications have led to numerous technological advancements. One of the most common applications of freezing is refrigeration. By leveraging the freezing process, we can preserve food, medicines, and other perishable items for extended periods, preventing spoilage and maintaining their quality. The development of cryogenics, a branch of physics that deals with extremely low temperatures, has also been made possible by understanding freezing principles. Cryogenic applications range from medical procedures, such as freezing and storing human embryos, to cutting-edge technologies like superconductivity.Freezing is also utilized in various manufacturing processes. Quick freezing techniques, such as flash freezing and blastfreezing, are employed in the food industry to preserve the nutritional value and taste of frozen products. In metallurgy, the controlled cooling of metals allows for the formation of desired structures, enhancing their strength and durability.Daily Life and Freeze:Beyond scientific and industrial applications, freeze affects our daily lives in numerous ways. During winter months, freezing temperatures can cause transportation disruptions, school closures, and inconvenience to daily commutes. The formation of ice on roads and walkways poses safety risks, necessitating the use of de-icing agents and precautions to prevent accidents. Additionally, winter sports enthusiasts eagerly await the freezing of lakes and slopes to pursue activities like ice fishing, ice skating, and skiing.Conclusion:The phenomenon of freeze is not only captivating from a scientific standpoint but also holds significant implications for our environment, technology, and everyday life. From its role in shaping ecosystems and climate patterns to its applications in refrigeration, cryogenics, and manufacturing processes, freezing is deeply intertwined with various aspects of humancivilization. By studying freeze and its impacts, we can better understand and appreciate the profound influence it has on our world.。
高温超导体的红外光学响应

高温超导体的红外光学响应英文回答:High-temperature superconductors (HTS) are materials that exhibit superconductivity at relatively high temperatures, compared to traditional superconductors. These materials have a wide range of applications, including in the field of infrared (IR) optics. The IR optical response of HTS materials is of great interest due to their potential use in various devices and systems.The IR optical response of HTS materials refers to how these materials interact with infrared light. This interaction can be characterized by various parameters, such as reflectivity, transmittance, and absorbance. These parameters determine how much of the incident IR light is reflected, transmitted, or absorbed by the HTS material.One important aspect of the IR optical response of HTS materials is their reflectivity. Reflectivity is a measureof how much of the incident IR light is reflected by the material. In the case of HTS materials, their reflectivity can be influenced by factors such as the material's crystal structure, composition, and surface quality. For example, a smooth and polished surface of an HTS material may have higher reflectivity compared to a rough or oxidized surface.Another important parameter is transmittance, which refers to the fraction of incident IR light that passes through the material. The transmittance of HTS materialscan be affected by factors such as the material's thickness, impurities, and defects. For instance, a thicker HTSmaterial may have lower transmittance compared to a thinner one due to increased absorption and scattering of the IR light.Absorbance is a measure of how much of the incident IR light is absorbed by the material. The absorbance of HTS materials can be influenced by factors such as thematerial's energy gap, impurity levels, and temperature.For example, at certain IR frequencies, HTS materials may exhibit strong absorbance due to their energy gap matchingthe energy of the incident light.Understanding the IR optical response of HTS materialsis crucial for the design and optimization of devices and systems that utilize these materials. For example, in the field of IR detectors, the IR optical response of HTS materials can affect their sensitivity and performance. By studying and manipulating the IR optical response, researchers can develop HTS-based devices with enhanced performance and capabilities.中文回答:高温超导体(HTS)是相对于传统超导体而言,在相对较高的温度下表现出超导性的材料。
自动化控制工程外文翻译外文文献英文文献

Team-Centered Perspective for Adaptive Automation DesignLawrence J.PrinzelLangley Research Center, Hampton, VirginiaAbstractAutomation represents a very active area of human factors research. Thejournal, Human Factors, published a special issue on automation in 1985.Since then, hundreds of scientific studies have been published examiningthe nature of automation and its interaction with human performance.However, despite a dramatic increase in research investigating humanfactors issues in aviation automation, there remain areas that need furtherexploration. This NASA Technical Memorandum describes a new area ofIt discussesautomation design and research, called “adaptive automation.” the concepts and outlines the human factors issues associated with the newmethod of adaptive function allocation. The primary focus is onhuman-centered design, and specifically on ensuring that adaptiveautomation is from a team-centered perspective. The document showsthat adaptive automation has many human factors issues common totraditional automation design. Much like the introduction of other new technologies and paradigm shifts, adaptive automation presents an opportunity to remediate current problems but poses new ones forhuman-automation interaction in aerospace operations. The review here isintended to communicate the philosophical perspective and direction ofadaptive automation research conducted under the Aerospace OperationsSystems (AOS), Physiological and Psychological Stressors and Factors (PPSF)project.Key words:Adaptive Automation; Human-Centered Design; Automation;Human FactorsIntroduction"During the 1970s and early 1980s...the concept of automating as much as possible was considered appropriate. The expected benefit was a reduction inpilot workload and increased safety...Although many of these benefits have beenrealized, serious questions have arisen and incidents/accidents that have occurredwhich question the underlying assumptions that a maximum availableautomation is ALWAYS appropriate or that we understand how to designautomated systems so that they are fully compatible with the capabilities andlimitations of the humans in the system."---- ATA, 1989The Air Transport Association of America (ATA) Flight Systems Integration Committee(1989) made the above statement in response to the proliferation of automation in aviation. They noted that technology improvements, such as the ground proximity warning system, have had dramatic benefits; others, such as the electronic library system, offer marginal benefits at best. Such observations have led many in the human factors community, most notably Charles Billings (1991; 1997) of NASA, to assert that automation should be approached from a "human-centered design" perspective.The period from 1970 to the present was marked by an increase in the use of electronic display units (EDUs); a period that Billings (1997) calls "information" and “management automation." The increased use of altitude, heading, power, and navigation displays; alerting and warning systems, such as the traffic alert and collision avoidance system (TCAS) and ground proximity warning system (GPWS; E-GPWS; TAWS); flight management systems (FMS) and flight guidance (e.g., autopilots; autothrottles) have "been accompanied by certain costs, including an increased cognitive burden on pilots, new information requirements that have required additional training, and more complex, tightly coupled, less observable systems" (Billings, 1997). As a result, human factors research in aviation has focused on the effects of information and management automation. The issues of interest include over-reliance on automation, "clumsy" automation (e.g., Wiener, 1989), digital versus analog control, skill degradation, crew coordination, and data overload (e.g., Billings, 1997). Furthermore, research has also been directed toward situational awareness (mode & state awareness; Endsley, 1994; Woods & Sarter, 1991) associated with complexity, coupling, autonomy, and inadequate feedback. Finally, human factors research has introduced new automation concepts that will need to be integrated into the existing suite of aviationautomation.Clearly, the human factors issues of automation have significant implications for safetyin aviation. However, what exactly do we mean by automation? The way we choose to define automation has considerable meaning for how we see the human role in modern aerospace s ystems. The next section considers the concept of automation, followed by an examination of human factors issues of human-automation interaction in aviation. Next, a potential remedy to the problems raised is described, called adaptive automation. Finally, the human-centered design philosophy is discussed and proposals are made for how the philosophy can be applied to this advanced form of automation. The perspective is considered in terms of the Physiological /Psychological Stressors & Factors project and directions for research on adaptive automation.Automation in Modern AviationDefinition.Automation refers to "...systems or methods in which many of the processes of production are automatically performed or controlled by autonomous machines or electronic devices" (Parsons, 1985). Automation is a tool, or resource, that the human operator can use to perform some task that would be difficult or impossible without machine aiding (Billings, 1997). Therefore, automation can be thought of as a process of substituting the activity of some device or machine for some human activity; or it can be thought of as a state of technological development (Parsons, 1985). However, some people (e.g., Woods, 1996) have questioned whether automation should be viewed as a substitution of one agent for another (see "apparent simplicity, real complexity" below). Nevertheless, the presence of automation has pervaded almost every aspect of modern lives. From the wheel to the modern jet aircraft, humans have sought to improve the quality of life. We have built machines and systems that not only make work easier, more efficient, and safe, but also give us more leisure time. The advent of automation has further enabled us to achieve this end. With automation, machines can now perform many of the activities that we once had to do. Our automobile transmission will shift gears for us. Our airplanes will fly themselves for us. All we have to dois turn the machine on and off. It has even been suggested that one day there may not be aaccidents resulting from need for us to do even that. However, the increase in “cognitive” faulty human-automation interaction have led many in the human factors community to conclude that such a statement may be premature.Automation Accidents. A number of aviation accidents and incidents have been directly attributed to automation. Examples of such in aviation mishaps include (from Billings, 1997):DC-10 landing in control wheel steering A330 accident at ToulouseB-747 upset over Pacific DC-10 overrun at JFK, New YorkB-747 uncommandedroll,Nakina,Ont. A320 accident at Mulhouse-HabsheimA320 accident at Strasbourg A300 accident at NagoyaB-757 accident at Cali, Columbia A320 accident at BangaloreA320 landing at Hong Kong B-737 wet runway overrunsA320 overrun at Warsaw B-757 climbout at ManchesterA310 approach at Orly DC-9 wind shear at CharlotteBillings (1997) notes that each of these accidents has a different etiology, and that human factors investigation of causes show the matter to be complex. However, what is clear is that the percentage of accident causes has fundamentally shifted from machine-caused to human-caused (estimations of 60-80% due to human error) etiologies, and the shift is attributable to the change in types of automation that have evolved in aviation.Types of AutomationThere are a number of different types of automation and the descriptions of them vary considerably. Billings (1997) offers the following types of automation:?Open-Loop Mechanical or Electronic Control.Automation is controlled by gravity or spring motors driving gears and cams that allow continous and repetitive motion. Positioning, forcing, and timing were dictated by the mechanism and environmental factors (e.g., wind). The automation of factories during the Industrial Revolution would represent this type of automation.?Classic Linear Feedback Control.Automation is controlled as a function of differences between a reference setting of desired output and the actual output. Changes a re made to system parameters to re-set the automation to conformance. An example of this type of automation would be flyball governor on the steam engine. What engineers call conventional proportional-integral-derivative (PID) control would also fit in this category of automation.?Optimal Control. A computer-based model of controlled processes i s driven by the same control inputs as that used to control the automated process. T he model output is used to project future states and is thus used to determine the next control input. A "Kalman filtering" approach is used to estimate the system state to determine what the best control input should be.?Adaptive Control. This type of automation actually represents a number of approaches to controlling automation, but usually stands for automation that changes dynamically in response to a change in state. Examples include the use of "crisp" and "fuzzy" controllers, neural networks, dynamic control, and many other nonlinear methods.Levels of AutomationIn addition to “types ” of automation, we can also conceptualize different “levels ” of automation control that the operator can have. A number of taxonomies have been put forth, but perhaps the best known is the one proposed by Tom Sheridan of Massachusetts Institute of Technology (MIT). Sheridan (1987) listed 10 levels of automation control:1. The computer offers no assistance, the human must do it all2. The computer offers a complete set of action alternatives3. The computer narrows the selection down to a few4. The computer suggests a selection, and5. Executes that suggestion if the human approves, or6. Allows the human a restricted time to veto before automatic execution, or7. Executes automatically, then necessarily informs the human, or8. Informs the human after execution only if he asks, or9. Informs the human after execution if it, the computer, decides to10. The computer decides everything and acts autonomously, ignoring the humanThe list covers the automation gamut from fully manual to fully automatic. Although different researchers define adaptive automation differently across these levels, the consensus is that adaptive automation can represent anything from Level 3 to Level 9. However, what makes adaptive automation different is the philosophy of the approach taken to initiate adaptive function allocation and how such an approach may address t he impact of current automation technology.Impact of Automation TechnologyAdvantages of Automation . Wiener (1980; 1989) noted a number of advantages to automating human-machine systems. These include increased capacity and productivity, reduction of small errors, reduction of manual workload and mental fatigue, relief from routine operations, more precise handling of routine operations, economical use of machines, and decrease of performance variation due to individual differences. Wiener and Curry (1980) listed eight reasons for the increase in flight-deck automation: (a) Increase in available technology, such as FMS, Ground Proximity Warning System (GPWS), Traffic Alert andCollision Avoidance System (TCAS), etc.; (b) concern for safety; (c) economy, maintenance, and reliability; (d) workload reduction and two-pilot transport aircraft certification; (e) flight maneuvers and navigation precision; (f) display flexibility; (g) economy of cockpit space; and (h) special requirements for military missions.Disadvantages o f Automation. Automation also has a number of disadvantages that have been noted. Automation increases the burdens and complexities for those responsible for operating, troubleshooting, and managing systems. Woods (1996) stated that automation is "...a wrapped package -- a package that consists of many different dimensions bundled together as a hardware/software system. When new automated systems are introduced into a field of practice, change is precipitated along multiple dimensions." As Woods (1996) noted, some of these changes include: ( a) adds to or changes the task, such as device setup and initialization, configuration control, and operating sequences; (b) changes cognitive demands, such as requirements for increased situational awareness; (c) changes the roles of people in the system, often relegating people to supervisory controllers; (d) automation increases coupling and integration among parts of a system often resulting in data overload and "transparency"; and (e) the adverse impacts of automation is often not appreciated by those who advocate the technology. These changes can result in lower job satisfaction (automation seen as dehumanizing human roles), lowered vigilance, fault-intolerant systems, silent failures, an increase in cognitive workload, automation-induced failures, over-reliance, complacency, decreased trust, manual skill erosion, false alarms, and a decrease in mode awareness (Wiener, 1989).Adaptive AutomationDisadvantages of automation have resulted in increased interest in advanced automation concepts. One of these concepts is automation that is dynamic or adaptive in nature (Hancock & Chignell, 1987; Morrison, Gluckman, & Deaton, 1991; Rouse, 1977; 1988). In an aviation context, adaptive automation control of tasks can be passed back and forth between the pilot and automated systems in response to the changing task demands of modern aircraft. Consequently, this allows for the restructuring of the task environment based upon (a) what is automated, (b) when it should be automated, and (c) how it is automated (Rouse, 1988; Scerbo, 1996). Rouse(1988) described criteria for adaptive aiding systems:The level of aiding, as well as the ways in which human and aidinteract, should change as task demands vary. More specifically,the level of aiding should increase as task demands become suchthat human performance will unacceptably degrade withoutaiding. Further, the ways in which human and aid interact shouldbecome increasingly streamlined as task demands increase.Finally, it is quite likely that variations in level of aiding andmodes of interaction will have to be initiated by the aid rather thanby the human whose excess task demands have created a situationrequiring aiding. The term adaptive aiding is used to denote aidingconcepts that meet [these] requirements.Adaptive aiding attempts to optimize the allocation of tasks by creating a mechanism for determining when tasks need to be automated (Morrison, Cohen, & Gluckman, 1993). In adaptive automation, the level or mode of automation can be modified in real time. Further, unlike traditional forms of automation, both the system and the pilot share control over changes in the state of automation (Scerbo, 1994; 1996). Parasuraman, Bahri, Deaton, Morrison, and Barnes (1992) have argued that adaptive automation represents the optimal coupling of the level of pilot workload to the level of automation in the tasks. Thus, adaptive automation invokes automation only when task demands exceed the pilot's capabilities. Otherwise, the pilot retains manual control of the system functions. Although concerns have been raised about the dangers of adaptive automation (Billings & Woods, 1994; Wiener, 1989), it promises to regulate workload, bolster situational awareness, enhance vigilance, maintain manual skill levels, increase task involvement, and generally improve pilot performance.Strategies for Invoking AutomationPerhaps the most critical challenge facing system designers seeking to implement automation concerns how changes among modes or levels of automation will be accomplished (Parasuraman e t al., 1992; Scerbo, 1996). Traditional forms of automation usually start with some task or functional analysis and attempt to fit the operational tasks necessary to the abilities of the human or the system. The approach often takes the form of a functional allocation analysis (e.g., Fitt's List) in which an attempt is made to determine whether the human or the system is better suited to do each task. However, many in the field have pointed out the problem with trying to equate the two in automated systems, as each have special characteristics that impede simple classification taxonomies. Such ideas as these have led some to suggest other ways of determining human-automation mixes. Although certainly not exhaustive, some of these ideas are presented below.Dynamic Workload Assessment.One approach involves the dynamic assessment o fmeasures t hat index the operators' state of mental engagement. (Parasuraman e t al., 1992; Rouse,1988). The question, however, is what the "trigger" should be for the allocation of functions between the pilot and the automation system. Numerous researchers have suggested that adaptive systems respond to variations in operator workload (Hancock & Chignell, 1987; 1988; Hancock, Chignell & Lowenthal, 1985; Humphrey & Kramer, 1994; Reising, 1985; Riley, 1985; Rouse, 1977), and that measures o f workload be used to initiate changes in automation modes. Such measures include primary and secondary-task measures, subjective workload measures, a nd physiological measures. T he question, however, is what adaptive mechanism should be used to determine operator mental workload (Scerbo, 1996).Performance Measures. One criterion would be to monitor the performance of the operator (Hancock & Chignel, 1987). Some criteria for performance would be specified in the system parameters, and the degree to which the operator deviates from the criteria (i.e., errors), the system would invoke levels of adaptive automation. For example, Kaber, Prinzel, Clammann, & Wright (2002) used secondary task measures to invoke adaptive automation to help with information processing of air traffic controllers. As Scerbo (1996) noted, however,"...such an approach would be of limited utility because the system would be entirely reactive."Psychophysiological M easures.Another criterion would be the cognitive and attentional state of the operator as measured by psychophysiological measures (Byrne & Parasuraman, 1996). An example of such an approach is that by Pope, Bogart, and Bartolome (1996) and Prinzel, Freeman, Scerbo, Mikulka, and Pope (2000) who used a closed-loop system to dynamically regulate the level of "engagement" that the subject had with a tracking task. The system indexes engagement on the basis of EEG brainwave patterns.Human Performance Modeling.Another approach would be to model the performance of the operator. The approach would allow the system to develop a number of standards for operator performance that are derived from models of the operator. An example is Card, Moran, and Newell (1987) discussion of a "model human processor." They discussed aspects of the human processor that could be used to model various levels of human performance. Another example is Geddes (1985) and his colleagues (Rouse, Geddes, & Curry, 1987-1988) who provided a model to invoke automation based upon system information, the environment, and expected operator behaviors (Scerbo, 1996).Mission Analysis. A final strategy would be to monitor the activities of the mission or task (Morrison & Gluckman, 1994). Although this method of adaptive automation may be themost accessible at the current state of technology, Bahri et al. (1992) stated that such monitoring systems lack sophistication and are not well integrated and coupled to monitor operator workload or performance (Scerbo, 1996). An example of a mission analysis approach to adaptive automation is Barnes and Grossman (1985) who developed a system that uses critical events to allocate among automation modes. In this system, the detection of critical events, such as emergency situations or high workload periods, invoked automation.Adaptive Automation Human Factors IssuesA number of issues, however, have been raised by the use of adaptive automation, and many of these issues are the same as those raised almost 20 years ago by Curry and Wiener (1980). Therefore, these issues are applicable not only to advanced automation concepts, such as adaptive automation, but to traditional forms of automation already in place in complex systems (e.g., airplanes, trains, process control).Although certainly one can make the case that adaptive automation is "dressed up" automation and therefore has many of the same problems, it is also important to note that the trend towards such forms of automation does have unique issues that accompany it. As Billings & Woods (1994) stated, "[i]n high-risk, dynamic environments...technology-centered automation has tended to decrease human involvement in system tasks, and has thus impaired human situation awareness; both are unwanted consequences of today's system designs, but both are dangerous in high-risk systems. [At its present state of development,] adaptive ("self-adapting") automation represents a potentially serious threat ... to the authority that the human pilot must have to fulfill his or her responsibility for flight safety."The Need for Human Factors Research.Nevertheless, such concerns should not preclude us from researching the impact that such forms of advanced automation are sure to have on human performance. Consider Hancock’s (1996; 1997) examination of the "teleology for technology." He suggests that automation shall continue to impact our lives requiring humans to co-evolve with the technology; Hancock called this "techneology."What Peter Hancock attempts to communicate to the human factors community is that automation will continue to evolve whether or not human factors chooses to be part of it. As Wiener and Curry (1980) conclude: "The rapid pace of automation is outstripping one's ability to comprehend all the implications for crew performance. It is unrealistic to call for a halt to cockpit automation until the manifestations are completely understood. We do, however, call for those designing, analyzing, and installing automatic systems in the cockpit to do so carefully; to recognize the behavioral effects of automation; to avail themselves of present andfuture guidelines; and to be watchful for symptoms that might appear in training andoperational settings." The concerns they raised are as valid today as they were 23 years ago.However, this should not be taken to mean that we should capitulate. Instead, becauseobservation suggests that it may be impossible to fully research any new Wiener and Curry’stechnology before implementation, we need to form a taxonomy and research plan tomaximize human factors input for concurrent engineering of adaptive automation.Classification of Human Factors Issues. Kantowitz and Campbell (1996)identified some of the key human factors issues to be considered in the design of advancedautomated systems. These include allocation of function, stimulus-response compatibility, andmental models. Scerbo (1996) further suggested the need for research on teams,communication, and training and practice in adaptive automated systems design. The impactof adaptive automation systems on monitoring behavior, situational awareness, skilldegradation, and social dynamics also needs to be investigated. Generally however, Billings(1997) stated that the problems of automation share one or more of the followingcharacteristics: Brittleness, opacity, literalism, clumsiness, monitoring requirement, and dataoverload. These characteristics should inform design guidelines for the development, analysis,and implementation of adaptive automation technologies. The characteristics are defined as: ?Brittleness refers to "...an attribute of a system that works well under normal or usual conditions but that does not have desired behavior at or close to some margin of its operating envelope."?Opacity reflects the degree of understanding of how and why automation functions as it does. The term is closely associated with "mode awareness" (Sarter & Woods, 1994), "transparency"; or "virtuality" (Schneiderman, 1992).?Literalism concern the "narrow-mindedness" of the automated system; that is, theflexibility of the system to respond to novel events.?Clumsiness was coined by Wiener (1989) to refer to automation that reduced workload demands when the demands are already low (e.g., transit flight phase), but increases them when attention and resources are needed elsewhere (e.g., descent phase of flight). An example is when the co-pilot needs to re-program the FMS, to change the plane's descent path, at a time when the co-pilot should be scanning for other planes.?Monitoring requirement refers to the behavioral and cognitive costs associated withincreased "supervisory control" (Sheridan, 1987; 1991).?Data overload points to the increase in information in modern automated contexts (Billings, 1997).These characteristics of automation have relevance for defining the scope of humanfactors issues likely to plague adaptive automation design if significant attention is notdirected toward ensuring human-centered design. The human factors research communityhas noted that these characteristics can lead to human factors issues of allocation of function(i.e., when and how should functions be allocated adaptively); stimulus-response compatibility and new error modes; how adaptive automation will affect mental models,situation models, and representational models; concerns about mode unawareness and-of-the-loop” performance problem; situation awareness decay; manual skill decay and the “outclumsy automation and task/workload management; and issues related to the design of automation. This last issue points to the significant concern in the human factors communityof how to design adaptive automation so that it reflects what has been called “team-centered”;that is, successful adaptive automation will l ikely embody the concept of the “electronic team member”. However, past research (e.g., Pilots Associate Program) has shown that designing automation to reflect such a role has significantly different requirements than those arising in traditional automation design. The field is currently focused on answering the questions,does that definition translate into“what is it that defines one as a team member?” and “howUnfortunately, the literature also shows that the designing automation to reflect that role?” answer is not transparent and, therefore, adaptive automation must first tackle its own uniqueand difficult problems before it may be considered a viable prescription to currenthuman-automation interaction problems. The next section describes the concept of the electronic team member and then discusses t he literature with regard to team dynamics, coordination, communication, shared mental models, and the implications of these foradaptive automation design.Adaptive Automation as Electronic Team MemberLayton, Smith, and McCoy (1994) stated that the design of automated systems should befrom a team-centered approach; the design should allow for the coordination betweenmachine agents and human practitioners. However, many researchers have noted that automated systems tend to fail as team players (Billings, 1991; Malin & Schreckenghost,1992; Malin et al., 1991;Sarter & Woods, 1994; Scerbo, 1994; 1996; Woods, 1996). Thereason is what Woods (1996) calls “apparent simplicity, real complexity.”Apparent Simplicity, Real Complexity.Woods (1996) stated that conventional wisdomabout automation makes technology change seem simple. Automation can be seen as simply changing the human agent for a machine agent. Automation further provides for more optionsand methods, frees up operator time to do other things, provides new computer graphics and interfaces, and reduces human error. However, the reality is that technology change has often。
几乎一致的临界电流密度 英文

几乎一致的临界电流密度英文English:In superconductivity, the nearly identical critical current density refers to the phenomenon where the critical current density of a material is almost the same in different orientations and magnetic fields. This is a crucial characteristic for practical applications, as it ensures the consistent performance of superconducting materials in various operating conditions. The nearly identical critical current density is a result of the strong pinning of magnetic flux lines in the material, which prevents them from moving and causing the superconducting state to break down. This property allows superconducting materials to maintain their superconducting properties over a wide range of external factors, making them valuable for applications such as magnets, power transmission lines, and medical imaging devices.中文翻译:在超导性中,几乎一致的临界电流密度指的是材料在不同方向和磁场下的临界电流密度几乎相同的现象。
控制万物的芯片作文

控制万物的芯片作文英文回答:Controlling everything with a chip is a concept that has fascinated scientists and technologists for decades. The idea of having a tiny, powerful chip that can control and monitor every aspect of our lives is both exciting and terrifying. On one hand, it promises convenience and efficiency, but on the other hand, it raises concerns about privacy and autonomy.Imagine a world where every device, every appliance, and even every individual is connected to a central chip. This chip would have the ability to collect and analyze data, make decisions, and communicate with other chips. It would be the ultimate control center, dictating how our homes, our cities, and even our bodies function.With such a chip, our lives would become incredibly convenient. Imagine waking up in the morning and havingyour chip automatically brew your favorite coffee, adjust the temperature in your house, and even select the perfect outfit for the day based on the weather forecast. Throughout the day, the chip would monitor your health, reminding you to exercise, eat healthy, and take breaks when needed. It would also handle mundane tasks like paying bills, scheduling appointments, and even driving your car.However, this level of control also raises concerns about privacy and autonomy. With a chip that knows everything about us, there is a risk of our personal information being accessed and misused. Moreover, if everything is controlled by a central chip, we may lose the ability to make decisions for ourselves. Our lives would become dictated by algorithms and data analysis, leaving little room for spontaneity and personal choice.Furthermore, the idea of a chip controlling everything also raises ethical questions. Who would have access tothis technology? Would it be available to everyone or only to those who can afford it? And what would happen if the chip malfunctions or falls into the wrong hands? Thepotential for abuse and manipulation is significant.In conclusion, the concept of controlling everything with a chip is both exciting and concerning. While it promises convenience and efficiency, it also raises concerns about privacy, autonomy, and ethical implications. As technology continues to advance, it is crucial to carefully consider the potential consequences and ensure that any implementation of such a chip is done in a waythat respects individual rights and values.中文回答:控制万物的芯片是一个让科学家和技术专家们着迷的概念,数十年来一直如此。
Vertex Corrections in the Spin-fluctuation-induced Superconductivity

a r X i v :c o n d -m a t /9910154v 1 [c o n d -m a t .s u p r -c o n ] 11 O c t 1999typeset using JPSJ.sty <ver.1.0b >Vertex Corrections in the Spin-fluctuation-induced SuperconductivityTakuya Okabe ∗Faculty of Engineering,Gunma University,Kiryu,Gunma 376-8515.(Received June 28,1999)We evaluate vertex corrections to T c on the basis of the antiferromagnetic spin-fluctuation model of the high-T c superconductivity.It is found that the corrections are attractive in the d x 2−y 2channel,and they become appreciable as we go through an intermediate-coupling regimeof T c ≃100K,the maximum T c attainable in the one-loop ´Eliashberg calculation.KEYWORDS:high-T c superconductor,antiferromagnetic spin fluctuation,vertex correctionAs a model for the high-T c superconductivity,the spinfluctuation mechanism has been one of the most widely discussed.1,2)The model assumes that quasiparticle is coupled with antiferromagnetic spin fluctuation,repre-sented by a peculiar low-energy expression for the mag-netic susceptibility.1,3)The phenomenological coupling constant to fit the transition temperature T c is used to explain,among others,the anomalous transport prop-erties consistently.1,4,5,6,7,8,9,10,11,12,13)On the other side,from a microscopic point of view,numerical studies based on the fluctuation exchange (FLEX)approxima-tion 14)have been carried out by many authors to esti-mate T c as well as to explain the deviations from the normal Fermi liquid behavior.14,15,16,17)Computational feasibility of these strong coupling theories rests on the effective use of a fast Fourier transform (FFT)algorithm.To asses the quantitative aspect of the theories,correc-tions coming from higher-order terms are investigated for the vertex function at some fixed external momenta,e.g.,on the basis of the spin-fluctuation model,and the qualitative features of the effect are emerging to some extent.18,19,20,21,22,23,24)However,the total effect of the vertex corrections on the physical observables is yet to be estimated numerically.Indeed to do this is gener-ally formidable because of the inapplicability of the FFT to a required additional sum on internal frequency and momentum.In this paper,we manage to evaluate the vertex corrections to T c ,and discuss questions of conver-gence of the formal perturbation theory with respect to the coupling constant.To put it concretely,the following investigation is based on the model of Monthoux and Pines (MP),4,6,7)in which the self-energy Σ(p ,i ωn )is determined as a self-consistent solution of the equations,Σ(p ,i ωn )=g2T∗E-mail:okabe@phys.eg.gunma-u.ac.jpwhereG (0)(p ,i ωn )=1ωq −i ω,(5)whereωq ≡ωsf (1+ξ2(q −Q )2),Q =(π,π),(6)for q x >0and q y >0.We assumeχ(q ,i νn )=−1i νn −ωd ω=2ν2n+ω2d ω(n =0)=χ(q ,ω=0).(n =0)(7)Here it is noted that a cutoffω0has to be artificiallyintroduced so as to meet the condition χ(q ,i νn )→1/ν2n as |νn |→∞.4)For eq.(5),the integral in eq.(7)is analytically evaluated;χ(q ,i νn )=2χQ ωsf /π|νn |−ωq tan −1ω0Ωp ′,n ′V (p ,i ωn ;p ′,i ωn ′)×|G (p ′,i ωn ′)|2Φ(p ′,i ωn ′),(9)where Φ(p ,i ωn )is the anomalous self-energy.The pair-ing potential V (p ,i ωn ;p ′,i ωn ′)readsV (p ,i ωn ;p ′,i ωn ′)=V (1)(p −p ′,i ωn −i ωn ′)+V (2)v (p ,i ωn ;p ′,i ωn ′)+V (2)c (p ,i ωn ;p ′,i ωn ′),(10)2Takuya OKABE whereV(1)(p−p′,iωn−iωn′)=g2χ(p−p′,iωn−iωn′).(11)The second and third terms in eq.(10)originate fromthe vertex corrections that we discuss below.As we are concerned about the d-wave instability,in-troducing a notationf(p)p ≡1Ω p,n G(0)(p,iωn)e+iωn0=2e(εp−µ(0))/T+1,(18)in which we assume n=0.75throughout this paper.The shiftδµin eq.(2)is adjusted in every iteration to assure δn=2TVertex Corrections in the Spin-fluctuation-induced Superconductivity 3Fig.3.The diagram (a)for the vertex corrected pairing potentialV (2)v and (b)for V (2)c .with g 2=0.41eV 2of MP.7)This is not due to the size of a square lattice,as we see in Fig.2,where T c is shown as a function of g 2.At T =T c =90K,the 16×16lattice is large enough for us to conclude g 2=0.57eV 2in the self-consistent calculation including the effect of Σ(p ,i ωn ).A close inspection indicates that the disagreement orig-inates in details of χ(q ,i νm ).In fact,we find g 2=0.34,smaller than g 2=0.41of MP,if we adopt the second line,instead of the third line,of eq.(7)for n =0too.This means that T c depends sensitively on how we prepare χ(q ,i νn )in the low-energy regime.This is complemen-tary to the above remark on the high-energy contribution to T c .As this quantitative difference is not of our primal concern either,deferring this problem,we choose to use our own definition,i.e.,χ(q ,i ωn )for n =0is specified separately by eq.(7).The qualitative results presented below are not affected by this choice.Now let us discuss how we evaluate the vertex cor-rection.The pairing potentials V (2)v (p ,i ωn ;p ′,i ωn ′)and V (2)c (p ,i ωn ;p ′,i ωn ′)including the vertex correction are diagrammatically represented by Fig.3(a)and Fig.3(b),respectively.These potentials at low frequencies ωn =ωn ′=πT (for n =n ′=0)are particularly studied by Monthoux.24)To see the effect on T c precisely,however,we have to evaluate the kernel K (2)i (i ωn ,i ωn ′),eq.(16),for a full set of the Matsubara frequencies ωn and ωn ′,then the kernel must be diagonalized.In effect,this is not practical at present.Thus,as a tractable method,we set up perturbation theory to evaluate the vertex cor-rections to the eigenvalue κof the kernel.We shall make effective use of the results ob-tainable by means of the FFT.Let us introducethe eigenfunction ¯Φ(1)(i ωn )for the largest eigen-value κ(1)of the kernel K (1)(i ωn ,i ωn ′);¯Φ(1)(i ωn )= n ′K(1)(i ωn ,i ωn ′)¯Φ(1)(i ωn ′)=κ(1)¯Φ(1)(i ωn ).Then the eigenvalue κincluding the vertex corrections is given byκ=κ(1)+κ(2)v +κ(2)c ,(20)whereκ(2)i=n,n ′¯Φ(1)(i ωn )K (2)i(i ωn ,i ωn ′)¯Φ(1)(i ωn ′).(21)We assume ¯Φ(1)(i ωn )is normalized.On physicalFig.4.T c as a function of g 2.Triangles;calculated without Σ(p ,i ωn ).Circles;including the effect of Σ(p ,i ωn ).Diamonds;including the effect of Σ(p ,i ωn )as well as the vertex corrections V (2)v and V (2)c .Two squares at T =90K and 45K are calculatedwith Σ(p ,i ωn )and V (2)c but without V (2)v .grounds,the norm of ¯Φ(1)(i ωn )decreases quite rapidly as |ωn |increases.Therefore,the sum over the Matsub-ara frequencies in eq.(21)is allowed to be restricted in a narrow region around (n,n ′)∼(0,0).In effect,we evaluate K (2)i (i ωn ,i ωn ′)for a 16×16mesh around the Fermi energy.Moreover,in the remainder of the paper,the results are calculated on a 16×16square lattice.Measured in terms of the weight ¯Φ(1)(i ωn ) 2,we find |ωn |≤15πT ¯Φ(1)(i ωn ) 2=0.98,0.91and 0.78at T =T c =90K,45K and 22K,respectively.Even at low T c ,the error involved is not appreciable,for the coupling constant itself is small there.As Fig.2shows,a 16×16mesh in the momentum space is large enough to grasp the qualitative features caused by the vertex corrections.To prepare V (2)i (p ,i ωn ;p ′,i ωn ′)is most time-consuming.Therefore,first we use the bare Green’s function G (0)(p ,i ωn )instead of G (p ,i ωn )to provide V (2)i (p ,i ωn ;p ′,i ωn ′).With V (2)i (p ,i ωn ;p ′,i ωn ′)pthus calculated beforehand and G (p ,i ωn )of the solution ofeqs.(1)and (2),we calculate K (2)i (i ωn ,i ωn ′)in eq.(16).Then,to evaluate κ(2)i ,eq.(21),is straightforward,and the critical coupling g 2at T c is determined.Results thus obtained are shown in Fig.4,where the triangles (with-out Σ(p ,i ωn ))and circles (with Σ(p ,i ωn ))denote the results without the vertex corrections.(See Fig.2).Thediamonds include the vertex correction V (2)v as well as V (2)c ,while only the effect of V (2)c is taken into account for the two squares at T =90K and 45K.Several points are noted from the figure.In the firstplace,both the effects of V (2)v and V (2)c are attractive on the whole,or enhances T c of the d -wave instability.The effect of V (2)c (Fig.3(b)),19)however,is negligibly small,as noted by Monthoux.24)On the other hand,theeffect of V (2)v (Fig.3(a))is prominent.In particular,it affects the result of MP,denoted by the circles interpo-lated with the solid line in Fig.4,that the maximum transition temperature attainable in this model is about4Takuya OKABE Fig.5.At T=90K,κ(1)andκ(1)+κ(2)are shown as a functionof g2.Squares,including only the effect of V(2)c,are overlappingwith circles to denoteκ(1)without the vertex corrections.100K.4)In fact,T c as a function of g2shows no signof saturation,and keeps increasing beyond200K whenthe vertex correction V(2)v is taken into account.In this regard,the vertex correction has an effect more than amere scale-up of the effective coupling constant g2.Next,the effect ofΣ(p,iωn)on V(2)i has to be inves-tigated.To this end,G(p,iωn)to meet eqs.(1)and(2)is used to evaluate V(2)i (p,iωn;p′,iωn′).The maximumeigenvalues calculated for T=90K are shown in Fig.5 as a function of g2.The effect ofΣ(p,iωn)is to weaken the vertex corrections.The effect,however,is not ap-preciable for T c=90K,as we see from Fig.5in which we see g2=0.36while we have g2=0.32in Fig.4 in the case including the vertex par-ing these with g2=0.57without the vertex corrections, we conclude that the correction due toΣ(p,iωn)in V(2)iis not important at least at T c=90K.In other words, if the coupling constant g should be evaluated to ac-count for T c,our result is that the vertex correction is not negligibly small at this temperature,22)at variance with previous results.21,23)The discrepancy may be due to a high-energy contribution included in our calcula-tion,or it is traced back to the abovefinding of a slightrenormalization effect on V(2)i .On the other hand,forT c=180K,wefind that g2=0.73in Fig.4is modified to g2=1.57.The large modification in this case is due toa large coupling constant to realize that high transitiontemperature.The results in this regime must be taken with care.To the extent that the vertex corrections that wefound for the pairing potentials are not negligible,the vertex corrections to eq.(1)should have to be investi-gated next.23)The latter effect onΣ(p,iωn)will reduce T c somewhat particularly through the pair propagator|G(p′,iωn′)|2in eq.(16),according to the above note, as a result of enhanced quasiparticle damping.There-fore,we will be ultimately led to a convergent result ofT c(g2),somewhere in between the dashed and solid linesof Fig.4.The results,however,would then indicate that T c≃100K is on the verge of practical applicability of this kind of perturbation theory in g2,as inferred from Fig.4.Note that,for us in this context,to suffer a smallcorrection is more important than tofind out a high T c. In summary,a result of this paper is presented inFig.4,though the result at high temperature is some-what modified as stated above.Applying perturbationtheory to the eigenvalue of the kernel K(iωn,iωn′),we estimated the vertex corrections to T c as a function of the coupling constant g2on the basis of the spin-fluctuation model of the high-T c superconductivity.Wefound that the effect of Fig.3(b)is numerically negligi-ble as far as the d x2−y2pairing instability is concerned, while Fig.3(a)enhances T c appreciably.For T c∼100K, the effect ofΣ(p,iωn)mainly comes in through the pair propagator|G(p′,iωn′)|2,dressing the vertex functions is not so important.In a strong-coupling regime at high temperatures,the vertex corrections become even qualitatively important,particularly in case where T c in the one-loop´Eliashberg calculation is substantially sup-pressed by lifetime effects.We would like to thank J.Igarashi,M.Takahashi,T. Nagao,T.Yamamoto and N.Ishimura for valuable dis-cussion.This work was supported by the Japan Society for the Promotion of Science for Young Scientists.0.20.40.60.81ω0 (eV)0.511.52g 2(e V 2)00.20.40.60.8g 2 (eV 2)50100150200T c (K )(a)p’-p’(b)00.20.40.60.81g 2(eV 2)50100150200T c (K)0.20.30.40.50.6g 2 (eV 2)0.60.811.21.4κκ(1)κ(1)+κ(2)T=90 K。
Chapter 7-Superconductor ceramics

BCS Theory of Superconductivity
The electron pairs have a slightly lower energy and leave an energy gap above them on the order of .001 eV which inhibits the kind of collision interactions which lead to ordinary resistivity. For temperatures such that the thermal energy is less than the band gap, the material exhibits zero resistivity. Bardeen, Cooper, and Schrieffer received the Nobel Prize in 1972 for the development of the theory of superconductivity.
The Critical Field
Researcher stated that the upper critical field of yttrium-barium-copper-oxide is 14 Tesla at liquid nitrogen temperature (77 degrees Kelvin) and at least 60 Tesla at liquid helium temperature. The similar rare earth ceramic oxide, thuliumbarium-copper-oxide, was reported to have a critical field of 36 Tesla at liquid nitrogen temperature and 100 Tesla or greater at liquid helium temperature.
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
Superconductivity controlled by the magnetic state of ferromagnetic nanoparticlesA.A. Fraerman 1,B.A. Gribkov 1, S.A. Gusev 1, E. Il’ichev 2, A.Yu. Klimov 1, Yu.N. Nozdrin 1,G.L. Pakhomov 1, V.V. Rogov 1, R. Stolz 2 and S.N. Vdovichev 11Institute for Physics of Microstructures RAS, GSP 105, 603950 Nizhny Novgorod, Russia2Institute for Physical High Technology, Jena, Germany(Dated: July 9, 2004)Novel hybrid superconductor/ferromagnetic particles structures are presented. Arrays of Co submicron particles were fabricated on overlap, edge type Josephson junctions and on a narrow Nb microbridge by means of high-resolution e-beam lithography. We observed a strong dependence between the magnetic state of the particles and the field-dependent critical I c (H) of these structures.PACS numbers: 74.50.+r, 75.75.+a, 75.60.-dINTRODUCTION Recent advances in microfabrication techniques have increased the interest in application of submicron sized patterned magnetic elements. The prospective usage of patterned ferromagnetic particles of submicron sizes is accounted for by the well defined local magnetic fields. In particular, magnetic dots arrays can be used as artificial pinning centers in a superconductor thin film. Experimental investigation of such a hybrid superconductor/ferromagnetic particles system was begun in the last decade from pioneer works of D. Givord et al. [1,2] and is underway currently, for example [3,4]. Transport properties of a superconducting layer may also assist in determining the magnetic states of a ferromagnetic particles array [2]. However, the magnetic mechanism of pinning in thin films works close to T c , when “natural” pinning forces are insignificant [4,5]. Another way for controlling the superconducting state of a filmby magnetic circuit is through modulating thesuperconducting order parameter and creatingweak links [6]. This device can work as asuperconductor switch.In this paper, we present three novel hybrid structures: weak links - Josephson junctions (overlap and edge type) and a narrow superconductor microbridge patterned by magnetic dots arrays. The influence of the particle magnetization distribution on the static field-dependent critical current I c (H) of these structures has been investigated. All measurements were done at temperatures well below the superconducting transition. EDGE-TYPE JOSEPSON JUNCTION Theory. We propose to use the influence of a stray magnetic field of nanoparticles on quantum interference in a Josephson junction. We model a real structure as an infinite strip of superconductor cut by a narrow slot, see Fig. 1. Assuming a sinusoidal current-phase relationship, the field-dependent total current I(H) of the junction is expressed as ∫∫=SH i c dS e j H I )()(ϕ,(),20pH H x +ΦΛ=∂∂πϕwhere ϕ is the phase differences, j c is the critical current density [7]. If the ferromagnetic particles are placed near the barrier, see Fig. 1, ϕ can be written as particle ext ϕϕϕ+=,where ϕext , ϕparticle are the phase differences due to the external magnetic field and the magnetic field of the particles, respectively. It means that the magnetic field of the particles can change the Fraunhofer diffraction pattern I c (H). We now assume that the spatial distribution of the Josephson phase difference ϕ(x) across the junction barrier can be written aswhere Λ is the effective thickness of the junction (Λ ≈ 2λL , λL is the London penetration depth), H is the external magnetic field, H p is the magnetic field induced by the magnetic particles, Φ0=hc/e=2⋅10-7Oe ⋅cm 2 is the magnetic flux quantum. In particular, if the magnetic moment of a single particle in the chain has a uniform distribution, H p (x ), ϕparticle are periodic functions. The results of numeric simulation of I c (H) for a junction with five dipoles is shown on Fig. 2a. In this case the field-dependent critical current should have amaximum when the magnetic flux per period of the array of nanoparticles at the Josephson junction contains an integer number of flux quanta Φd = n Φ0, (Φd = H Λd , n is integer). A more explicit model for this case confirmed the same result [8].Experiment. For experimental investigation of the dependence of the particles magnetization distribution on the critical current we fabricated a series of Nb\SiN x \Nb edge-type Josephson junctions [9] with chains of ferromagnetic Co nanoparticles with a typical lateral size of 300-600 nm and a height of 25 nm (see Fig. 1). Measurements of the critical current I c (H) were performed by a standard four-terminal method at T=4.2K , in the magnetic field normal to the plane of the junction.The particles were magnetized at room temperature either by applying a magnetic field of 20 kOe or by the MFM tip [10]. The magnetic state of the nanoparticles was controlled by MFM before and after the low temperature experiments.We present two series of experiments. 1). All particles were magnetized as dipoles (see inset on Fig. 2b) 2). All particles were magnetized close to the vortex state. In thecase of the dipole magnetic states, weFig. 1. Schematic and SEM image of an edge-type Josephson junction with a chain of magnetic nanoparticles.c maxima whose position depends on a period of the particles chain, as theory predicted, seeFig.2. In the case of the vortex magnetic states of the particles, the resonance effects were absent. This result is brought about by the weak magnetic field induced by the particles with a curling magnetization distribution.OVERLAP JOSEPHSON JUNCTIONThe basic idea of this experiment is to trap a regular lattice of the Abrikosov vortices in the top electrode of the overlap junction perpendicularly to the junction surface. This is a unique way for controlling the properties of the overlap type junction because neither the magnetic field induced by particles, nor the aligned vortices trapped in both electrodes affect the phase differences, i.e., properties of the junction. A special series of overlap geometry junctions Nb\Al\AlO x \Nb with a thin top electrode (30 nm) was produced by IPHT (Jene, Germany). The width and length of the junctions is about 20 µm . The top electrode thickness is smaller than λL of the Nb , whichvortices. The particles array was fabricated on the top of the junction, using electron beam lithography, see Fig. 3.Measurements of the critical current I c (H) were performed by the four-terminal method for helium temperature, in the magnetic field within the plane of the junction.It is evident that the trapping of vortices is controlled by the magnetic state of the particles. We also present two series ofFig. 3. SEM image of the overlap Josephson junction with an array of magnetic nanoparticles.experiments. 1). About 80% of the particles were magnetized as dipoles. 2). About 80% of the particles were magnetized as vortices. The Fraunhofer pattern for the vortex magnetic state of the particles and for junction without particles is the same, see Fig. 4a. The diffraction pattern for the dipole magnetic state of the dots has changed considerably, see Fig. 4b. The critical current has been suppressed; the form of the Fraunhofer pattern has changed and become different from zero at a high magnetic field. There are no resonance effects that can be explained by the absence of the regular vortex lattice. The results observed in this experiment slightly resemble those obtained in a special experiment for a single vortex motion [11].NARROW SUPERCONUCTORMICROBRIDGEWe fabricated a series of 1 µm wide Nb microbridges with the Nb thickness of about 0.1 µm (Тс~9 К) and formed a chain of Co nanoparticles with a typical lateral size of 300*600 nm and a height of 100 nm (Fig. 5a). The remanent magnetic state of the particles is inhomogeneous; particles can be magnetized to a uniform state by the field of 500-1000 Gs [13]. The density of the critical current for our Nb films is about 5 107 A/cm2, which implies deep centers of pinning [14].We performed standard measurements of the critical current I c(H) at T=4.2K in an external magnetic field normal to the plane of the microbridge.The critical current dramatically decreased with an increasing magnetic field and this dependence was the same for samples with and without particles. If a magnetic field is applied in the plane of a particle-free microbridge (up to 2.5 kOe), the critical current remains unaffected. For a microbridge with magnetic particles we observed theFig. 5. SEM image of microbridges with ferromagnetic nanoparticles.following effects, see Fig. 6: enhancement of the critical current I c to 20% with an increasing magnetic field H; strong dependence of the critical current on the magnetic field sign, the difference between I c(H) and I c(-H) reached 70% (“diode effect”). In addition, the critical current has a periodical component with a period about 500 Oe, see Fig. 6.Today we do not have a clear understanding of the mechanism behind the magnetic particles effect on the critical current of a microbridge, all existing hypotheses have some imperfections. It seems important, though, that our Nb film is polycrystalline and features a high density of the critical current, and the microbridge of our make is narrow, which determines the boundary condition for entrance of the vortices.CONCLUSIONWe investigated novel types of hybrid structures composed of ferromagnetic nanoparticles on superconductor, which can be used without control of the temperature. The transport properties of superconductors are well controlled by the magnetic state of the particles. For the case of the edge-type Josephson junction a simple model of the influence produced by magnetic particles is proposed. The experiments confirmed susceptibility of the Josephson junctions to the magnetic state of nanoparticles, which can be used in low temperature electronic devices.The experimental results obtained in the study of overlap geometry junction and narrow microbridge are novel, and there is no theory interpreting these effects nowadays. However, the observed effects are interesting both from the fundamental point of view and for prospective application.This work was supported by the RFBR, Grant number 03-02-16774, 04-02-16827, 04-02-17048 and INTAS, Grant number 03-51-6426, 03-51-4778.REFERENCE[1] Y. Otani, B. Pannetier, J.P. Nozieres, D. Givord. J. Magn. Magn. Mater. 126 (1993) 622. [2] O. Geoffroy, D. Givord, Y. Otany et al. J. Magn. Magn. Mater. 121 (1993) 223.[3] J.I. Martin, M. Velez, J. Nogues and I.K. Shuller. Phys. Rev. Lett. 79 (1997) 1929.[4] A.V. Silhanek, L. Van Look, S. Raedts et al. Phys. Rev. B 68 (2003) 214504.[5] I.D. Tokman. Phys. Let. A 166 (1992) 412.[6] T.W. Clinton, M. Johnson. J. Appl. Phys. 83 (1998) 6777.[7] A. Barone and G.Paterno. Phisics and Applications of the Josephson Effect (Wiley, New York, 1982; Mir, Moscow, 1984).[8] A.V. Samohkvalov. J. Exp. Tech. Phys. Lett. 78 (2003) 369.[9] S.N. Vdovichev, A.Yu. Klimov, Yu.N. Nozdrin, V.V. Rogov. Tech. Phys. Lett. 30 (2004).[10] A.A. Fraerman, B.A. Gribkov, S.A. Gusev et al. Low Dim. Struc. To be published.[11] O.B.Hyun, J.R.Clem, D.KJ.Finnemore. Phys.Rev. B 40 (1989) 175.[12] A. Alexeev. Private communication.[13] V.V. Schmidt. The physics of Superconductors.(MCNMO,Moscow,2000; Springer-Verlag, Berlin-Heidelberg, 1997).H, kOeI c, mAFig. 6. Dependence I c(H) of the microbridge with ferromagnetic nanoparticles.。
