Interacting electrons in disordered wires Anderson localization and low-temperature transpo

合集下载

Magnetic-field-dependent quasiparticle energy relaxation in mesoscopic wires

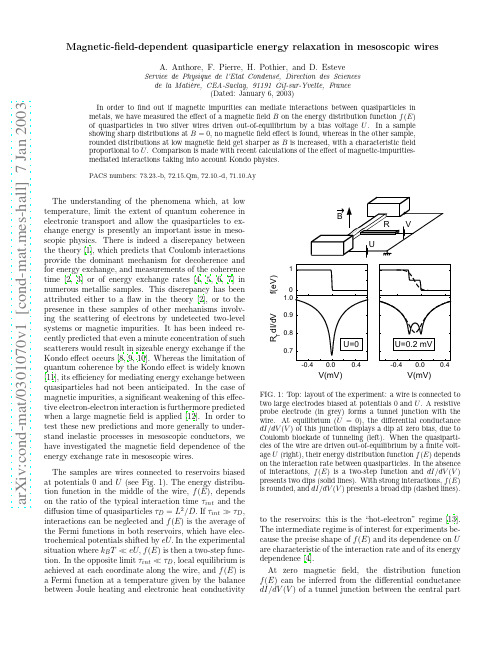
a r X i v :c o n d -m a t /0301070v 1 [c o n d -m a t .m e s -h a l l ] 7 J a n 2003Magnetic-ﬁeld-dependent quasiparticle energy relaxation in mesoscopic wiresA.Anthore,F.Pierre,H.Pothier,and D.EsteveService de Physique de l’Etat Condens´e ,Direction des Sciences de la Mati`e re,CEA-Saclay,91191Gif-sur-Yvette,France(Dated:January 6,2003)In order to ﬁnd out if magnetic impurities can mediate interactions between quasiparticles in metals,we have measured the eﬀect of a magnetic ﬁeld B on the energy distribution function f (E )of quasiparticles in two silver wires driven out-of-equilibrium by a bias voltage U .In a sample showing sharp distributions at B =0,no magnetic ﬁeld eﬀect is found,whereas in the other sample,rounded distributions at low magnetic ﬁeld get sharper as B is increased,with a characteristic ﬁeld proportional to U .Comparison is made with recent calculations of the eﬀect of magnetic-impurities-mediated interactions taking into account Kondo physics.PACS numbers:73.23.-b,72.15.Qm,72.10.-d,71.10.AyThe understanding of the phenomena which,at low temperature,limit the extent of quantum coherence in electronic transport and allow the quasiparticles to ex-change energy is presently an important issue in meso-scopic physics.There is indeed a discrepancy between the theory [1],which predicts that Coulomb interactions provide the dominant mechanism for decoherence and for energy exchange,and measurements of the coherence time [2,3]or of energy exchange rates [4,5,6,7]in numerous metallic samples.This discrepancy has been attributed either to a ﬂaw in the theory [2],or to the presence in these samples of other mechanisms involv-ing the scattering of electrons by undetected two-level systems or magnetic impurities.It has been indeed re-cently predicted that even a minute concentration of such scatterers would result in sizeable energy exchange if the Kondo eﬀect occurs [8,9,10].Whereas the limitation of quantum coherence by the Kondo eﬀect is widely known [11],its eﬃciency for mediating energy exchange between quasiparticles had not been anticipated.In the case of magnetic impurities,a signiﬁcant weakening of this eﬀec-tive electron-electron interaction is furthermore predicted when a large magnetic ﬁeld is applied [12].In order to test these new predictions and more generally to under-stand inelastic processes in mesoscopic conductors,we have investigated the magnetic ﬁeld dependence of the energy exchange rate in mesoscopic wires.The samples are wires connected to reservoirs biased at potentials 0and U (see Fig.1).The energy distribu-tion function in the middle of the wire,f (E ),depends on the ratio of the typical interaction time τint and the diﬀusion time of quasiparticles τD =L 2/D.If τint ≫τD ,interactions can be neglected and f (E )is the average of the Fermi functions in both reservoirs,which have elec-trochemical potentials shifted by eU.In the experimental situation where k B T ≪eU,f (E )is then a two-step func-tion.In the opposite limit τint ≪τD ,local equilibrium is achieved at each coordinate along the wire,and f (E )is a Fermi function at a temperature given by the balance between Joule heating and electronic heat conductivityT d I /d VRV(mV)V(mV)f (e V )FIG.1:Top:layout of the experiment:a wire is connected totwo large electrodes biased at potentials 0and U .A resistive probe electrode (in grey)forms a tunnel junction with the wire.At equilibrium (U =0),the diﬀerential conductance dI/dV (V )of this junction displays a dip at zero bias,due to Coulomb blockade of tunneling (left).When the quasiparti-cles of the wire are driven out-of-equilibrium by a ﬁnite volt-age U (right),their energy distribution function f (E )depends on the interaction rate between quasiparticles.In the absence of interactions,f (E )is a two-step function and dI/dV (V )presents two dips (solid lines).With strong interactions,f (E )is rounded,and dI/dV (V )presents a broad dip (dashed lines).to the reservoirs:this is the “hot-electron”regime [13].The intermediate regime is of interest for experiments be-cause the precise shape of f (E )and its dependence on U are characteristic of the interaction rate and of its energy dependence [4].At zero magnetic ﬁeld,the distribution function f (E )can be inferred from the diﬀerential conductance dI/dV (V )of a tunnel junction between the central partof the wire and a superconducting(aluminum)probe electrode biased at potential V[4].In magneticﬁelds larger than the criticalﬁeld B c∼0.1T of the supercon-ducting electrode,another method is required.Here,we have taken advantage of the nonlinearity of the current-voltage characteristic of a tunnel junction placed in series with a resistance R.When both electrodes of the junc-tion are in the normal state and at thermal equilibrium, the diﬀerential conductance dI/dV(V)displays a dip at V=0(see Fig.1),due to the Coulomb blockade of tun-neling[14].Assuming that the two electrodes have dif-ferent distribution functions f and f ref,the diﬀerential conductance reads:dIR TdE f(E) dεP(ε)(1)×∂2π e J(t)+iεt/ the probability for an electronto tunnel through the barrier while releasing to the envi-ronment an energyε,J(t)= dωR K e−iωt−1ω2Re[Z(ω)]V (mV)V (mV)R T d I /d VV (mV)FIG.3:Magnetic ﬁeld eﬀect in sample #1:diﬀerential con-ductance dI/dV (V )at U =0.1,0.2and 0.3mV,for B ranging (from bottom to top)from 0.3to 1.5T by steps of 0.3T.Suc-cessive curves have been vertically oﬀset by steps of 0.033,for clarity.at U =0.2mV ,and 1.5T at U =0.25mV (not shown),hence presenting a linear increase with U.The compari-son of the raw data on sample #1and sample #2in Fig.2already allows to conclude that sample #1presents an extra interaction which can be strongly reduced by ap-plying a magnetic ﬁeld.We now compare the experimental data with theoret-ical predictions.The distribution function is calculated by solving the stationary Boltzmann equation in the dif-fusive regime [17,18]:1∂x 2=−I in coll (x,E,{f })+I outcoll (x,E,{f })(2)where I in coll and I outcoll are the rates at which quasiparticles are scattered in and out of a state at energy E by inelastic processes.Assuming that the dominant inelastic process is a two-quasiparticle interaction which is local on the scale of variations of the distribution function,I in coll = d εd E ′K (ε)f x E +ε(1−f x E )f x E ′(1−f x E ′−ε)(3)where the shorthand f x E stands for f (x,E ).The out-collision term I outcoll has a similar form.The kernel func-tion K (ε)is proportional to the averaged squared in-teraction between two quasiparticles exchanging an en-ergy ε.Coulomb interactions lead,in diﬀusive wires,toK (ε)=κ/ε3/2[1],where κ=(π 2 3/2νF S )−1with νF the density of states at the Fermi level [19].The B =0data for sample #2can be well ﬁt using this term with κ=0.12ns −1meV −1/2,of the same order of magni-tude as the theoretical value 0.07ns −1meV −1/2[20],and a term of lesser importance describing phonon emission [21].The B =0data for sample #1can be ﬁt simi-larly,with κ=2.4ns −1meV −1/2,however the reduction of the energy exchange rate with B indicates that an extra process is present at B =0.We have in the fol-lowing ﬁxed κto the best ﬁt value obtained for the large ﬁeld,low U data,where the B −dependent interactionhas essentially vanished:κ=0.5ns −1meV −1/2[20].The remaining part of the energy exchange rate was ﬁt with the theory of G¨o ppert et al.[12,22],which accounts for the eﬀective interaction in the presence of a concentra-tion c of spin-14Cu samples,a material in which the oxyde at the sur-face of theﬁlms was found to cause dephasing at low temperature[24].Data on energy exchange[25]could also beﬁt with the theory of G¨o ppert et al[12],using T K=300mK,c=4.8ppm,g=2.3,νJ=0.1,on top ofa Coulombic term with intensityκ=0.5ns−1meV−1/2[22].This result gives evidence that the anomalous inter-actions observed in many Cu wires at B=0[4,7]are also due to magnetic impurities.Here also,measurements of the phase coherence time[7]are explained by signiﬁ-cantly smaller impurity concentrations(∼0.3ppm).This repeated discrepancy on the concentrations deduced from the two types of measurements remains an open problem. From an experimental point of view,a more quantitative test of theory could be obtained in samples with added, identiﬁed magnetic impurities at a known concentration [27].To conclude,we have found that anomalous energy exchange rates between quasiparticles were strongly re-duced by the application of a magneticﬁeld.Moreover, the energy and magneticﬁeld dependence of the exchange rate can be accurately accounted for by the presence of a small concentration of Kondo magnetic impurities[12]. It is worthwhile to compare this result with recent mea-surements on Aharonov-Bohm rings,which show that the small phase-coherence times found at B=0were in-creased in aﬁnite magneticﬁeld[28].All these measure-ments indicate that the presence of very dilute magnetic impurities is a very plausible candidate to explain both extra dephasing and extra energy exchange observed in many mesoscopic samples.We acknowledge the technical help of P.Orﬁla,fruit-ful discussions and correspondence with G.G¨o ppert,H. Grabert and N.Birge,and permanent input from M.De-voret,P.Joyez,C.Urbina and D.Vion.[1]For a review,see B.L.Altshuler and A.G.Aronov,in Electron-Electron Interactions in Disordered Systems, Ed.A.L.Efros and M.Pollak(Elsevier Science Publish-ers B.V.,1985).[2]P.Mohanty,E.M.Q.Jariwala and R.A.Webb,Phys.Rev.Lett.78,3366(1997).[3]For a review,see J.J.Lin and J.P.Bird,J.Phys.:Con-dens.Matter.14,R501(2002).[4]H.Pothier et al.,Phys.Rev.Lett.79,3490(1997).[5]F.Pierre et al.,J.Low Temp.Phys.118,437(2000).[6]F.Pierre et al.,in Kondo Eﬀect and Dephasing in Low-Dimensional Metallic Systems,Ed.V.Chandrasekhar,C.Van Haesendonck,and A.Zawadowski(Kluwer Aca-demic Publishers,Dordrecht,The Netherlands,2001), p.119(cond-mat/0012038).[7]F.Pierre,Ann.Phys.(Paris)26N4(2001).[8]A.Kaminski and L.I.Glazman,Phys.Rev.Lett.86,2400(2001).[9]G.G¨o ppert,H.Grabert,Phys.Rev.B64,033301(2001).[10]J.Kroha and A.Zawadowski,Phys.Rev.Lett.88,176803(2002).[11]C.Van Haesendonck,J.Vranken,Y.Bruynseraede,Phys.Rev.Lett.58,1968(1987).[12]G.G¨o ppert et al.,Phys.Rev.B66,195328(2002).[13]A.H.Steinbach,J.M.Martinis,and M.H.Devoret,Phys.Rev.Lett.76,3806(1996).[14]For a review,see G.-L.Ingold and Yu.Nazarov,in SingleCharge Tunneling,Ed.H.Grabert and M.H.Devoret (Plenum Press,New York,1992).[15]M.Tinkham,in Introduction to Superconductivity,Ed.Mc Graw-Hill(1985),section2-10.5.[16]Because the signal to noise ratio is not large enough,nu-merical deconvolution of the dI/dV(V)curves proved to be unreliable.In particular,the preliminary f(E)curves in[25]and in[12]were artiﬁcially rounded.We prefer to present here raw data.[17]V.I.Kozub and A.M.Rudin,Phys.Rev.B52,7853(1995).[18]K.E.Nagaev,Phys.Lett.A169,103(1992);Phys.Rev.B52,4740(1995).[19]A.Kamenev and A.Andreev,Phys.Rev.B60,2218(1999).[20]In other experiments with silver wires[5],theﬁt value ofκwas found to be between3and10times the theoretical one[7].This scatter in the intensity of the interaction is presently not understood.[21]F.Pierre et al.,Phys.Rev.Lett.86,1078(2001).Theamplitude of the electron-phonon interactions is set toa value deduced from weak localization data:κph=8ns−1meV−3.When taking this term into account,the data of[5]are wellﬁt by Coulomb interactions,see[7].A slight heating of the environment with U was also taken into account in theﬁts.[22]In Ref.[12],the agreement with experiment was poor fortwo reasons:ﬁrst,the deconvolved data are artiﬁcially rounded;second,the eﬀect of usual Coulombic interac-tions have not been taken into account in the calculation.[23]The dI/dV(V)are slightly asymetric because the mea-suring probe is out of the center of the wire by0.01L.Note that the increase of the interaction rate at inter-mediate magneticﬁelds[25],when the Zeeman splitting of the impurity states is smaller than eU,is not visible because f(E)is already close to a Fermi distribution at B=0.This experiment is much more sensitive to the decrease of the exchange rate,which leads to a sharper structure in f(E).[24]J.Vranken,C.Van Haesendonck,and Y.Bruynseraede,Phys.Rev.B37,8502(1988).[25]A.Anthore et al.,in Electronic Correlations:FromMeso-to Nano-Physics,Ed.T.Martin,G.Montam-baux and J.Trˆa n Thanh Vˆa n(EDP Sciences,2001) [cond-mat/0109297][26]M.B.Maple,Magnetism,edited by H.Suhl(Academic,New York,1973),Vol.5.[27]In practice,we have found that tunnel junctions with asilver electrode evolve,at room temperature,to an open circuit within a few hours,hence forbidding implantation of magnetic impurities.[28]F.Pierre and N.O.Birge,Phys.Rev.Lett.89,206804(2002).。

绝缘体与安德森绝缘体

Thouless’ picture
A very important step to modern theory
G e 2 g ( E F ) DLd 2
e 2 hD N ( EF ) 2 h L
dimensionless
Thouless energy
ET
hD h 2 L T
2 , is level spacing, 2 is for spin 2e 2 ET E G~ g~ T h N ( EF ) ~
Question: Is the presence of electronic band gap a necessary and/or sufficient condition for the formation of insulators?
Answer: It is neither necessary nor sufficient. Counter examples: Anderson insulators: insulating states without band gap (but with a mobility gap), so that it not necessary. Superconductors: there is a electronic band gap (superconducting gap) like insulators due to electron pairing, but current can flow without resistance. Since the systems support collective excitations beside single-electronic excitations. The super-current in the system is a collective excitations.

大三英语下翻译

Unit11. The devices,called defibrillators, fire an electrical shock to jolt the heart back into abnormalrhythm if it starts to beat in a disordered way that can cause sudden death.若心脏跳动失常，则有可能导致猝死。

这时，这种叫做心脏除颤器的装置，能电击心脏，使之恢复正常心律。

In people who truly need them ,for conditions that can fatally disrupt heart rhythm , defibrillators can be life-saving.对于那些确实需要除颤器的病人来说，这种疾病会使心脏节律遭受致命的破坏，除颤器可以挽救他们的生命。

2. Professional societies set guidelines that specify when the defibrillators should be used,based on studies showing which patients they help.基于除颤器对哪些患者有帮助的研究，职业协会制定操作指南，详细说明什么时候可以使用它。

To find out if doctors have been complying ,researchers examined the records of 111707 people who received the implants at 1227 hospitals in the United States from January 2006to June 2009 .The records were part of a national registry ,and the National Heart ,Lung and Blood Institute paid for the study .为了弄清楚医生是否在遵守这些指导方针，研究人员对111707名病人的医疗档案进行了检查，这些病人在2006年1月至2009年6月之间在美国的1227家医院植入了除颤器。

Direct-current nanogenerator driven by ultrasonic waves

Phys. Rev. Lett. 63, 2508 (1989). 4. T. J. Gramila, J. P. Eisenstein, A. H. MacDonald,
L. N. Pfeiffer, K. W. West, Phys. Rev. Lett. 66, 1216 (1991). 5. U. Sivan, P. M. Solomon, H. Shtrikman, Phys. Rev. Lett. 68, 1196 (1992). 6. M. P. Lilly, J. P. Eisenstein, L. N. Pfeiffer, K. W. West, Phys. Rev. Lett. 80, 1714 (1998). 7. J. G. S. Lok et al., Phys. Rev. B 63, 041305 (2001). 8. A. G. Rojo, J. Phys. Condens. Matter 11, R31 (1999). 9. D. Snoke, Science 298, 1368 (2002). 10. R. Pillarisetty et al., Phys. Rev. B 71, 115307 (2005). 11. M. Yamamoto, M. Stopa, Y. Tokura, Y. Hirayama, S. Tarucha, Science 313, 204 (2006). 12. B. N. Narozhny, I. L. Aleiner, Phys. Rev. Lett. 84, 5383 (2000). 13. N. A. Mortensen, K. Flensberg, A.-P. Jauho, Phys. Rev. B 65, 085317 (2002). 14. I. V. Lerner, Phys. Lett. A 133, 253 (1988).

博士自我介绍4篇

博士自我介绍4篇good morning, dear professors:it’s my honor to introduce myself. my name is xxx, i am fromxxcountyxxprovince, december xxxx i was born in a poor family, and my parents are farmers, i love and respect them very much. we were delight with my becoming a fresh man in september 1997. luckily, i was permitted to be a graduate student after 4 years colorful life on campus. i received my bachelor degreeXX inxx institute of science and technology, then a master degreeXX inxx university of science and technology. for those 7 years my major was die design. before i received my master degree, i had done the subject of xxxxxxxxxxx. for the past 3 years, i have been inxxxxxxcollege, where i have been and still am a teacher. i teach students machine design etc. i have published more than 10 first-author papers.自我介绍的第一句话，很关键，不用说的很复杂。

安德森先生的生平英文小作文

安德森先生的生平英文小作文Philip Anderson was born in Indianapolis and spent his childhood in Urbana, Illinois. He graduated from Urbana High School in 1940. He then went to Harvard University to complete his bachelor's degree and entered graduate school. Study physics under the tutelage of John Van Fleck. Anderson spent time at the U.S. Naval Research Laboratory during the war. From 1949 to 1984, Anderson worked at Bell Laboratories in New Jersey and extensively studied many problems in condensed matter physics. During this time he discovered the concept of Anderson localization; wrote the Anderson Hamiltonian to describe electrons in transition metal systems. In addition he advised particle physicists to look for the mechanism that produces the mass of particles (later called the Higgs mechanism); developed computational methods in the BCS theory of superconductors. In 1963, Anderson was elected to the American Academy of Arts and Sciences. Anderson was a professor of theoretical physics at the University of Cambridge from 1967 to 1975. In 1977 he was awarded the Nobel Prize in Physics together with Neville Mott and John Van Fleck for "fundamental theoreticalinvestigations of the electronic structure of magnetism and disordered systems". This research provided a theoretical basis for the technology of switching and memory in electronic components, and made important contributions to the later development of computers. In 1982 he received the National Medal of Science. Since 1984, he has retired from Bell Labs to chair the Joseph Henry Professor of Physics at Princeton University.。

电子显微镜检查在呼吸道感染病原学诊断中的意义

•6 •国际儿科学杂志2021年1月第48卷第1期Int J Pediatr.Jan 2021，Vol.48，No. 1•综述.电子显微镜检查在呼吸道感染病原学诊断中的意义汪亚南（综述）曹玲（审校）首都儿科研究所附属儿童医院呼吸内科，北京100020通信作者：曹玲，Email:caoling9919@ 163. com【摘要】电子显微镜技术在医学领域发挥着越来越重要的作用，常用于各系统的超微结构观察、机制研究和疾病的临床诊断等，其中在呼吸道病原学诊断中的作用不容忽视，但其相关的综述较少。

利用电子显微镜可以准确观察呼吸道病原体的形态学特征，并对新发呼吸道感染病原体进行初步的分类筛选。

但由于设备昂贵、技术难度大、操作复杂等因素，电子显微镜技术很少应用于常规的病原学诊断，而更多的用于实验室基础研究。

该文综述了电子显微镜检査在呼吸道病原学诊断方面的优缺点及意义。

【关键词】电子显微镜；诊断；呼吸道病原基金项目：北京市医院管理中心儿科学科协同发展中心专项经费资助项目（XTCX201821)DOI ：10.3760/cma.j.issn.1673-4408. 2021.01.002The significance of electron microscopy in the etiological diagnosis of respiratory tract infectionWang Yanan, Cao LingDepartment of Respiratory Medicine, Children's Hospital Affiliated to the Capital Institute o f Pediatrics, Beijing100020, ChinaCorresponding author：Cao Ling，Email：caoling9919@ 163. com【A bstract】At present，electron microscopy has played a more and more important role in the field ofmedicine. It is often used in the ultrastructure observation of various systems, mechanism research and clinicaldiagnosis of diseases. The role of electron microscopy in the etiological diagnosis of respiratory tract infectioncannot be ignored, but its related review is less. By using electron microscopy, the moqDhological characteristics of respiratory tract pathogens can be accurately observed and the new respiratory tract infection pathogenscan be classified and screened. In addition, through the combination of samples with patients' serum and observation of immuno-electron microscopy, the host response to pathogens can also be understood. However, dueto the high equipment requirements, technical difficulties，complex operation and other factors, electron microscopy is rarely used in conventional etiological diagnosis, and more used in laboratory basic research. In thispaper, the advantages, disadvantages and significance of electron microscopy in the etiological diagnosis of respiratory tract are reviewed.【Key words 】Electron microscopy; Diagnosis ；Respiratory tract pathogenFund program ：Special Fund Support Project of Pediatrics Collaborative Development Center of BeijingHospital Management Center (XTCX201821)DOI ：10. 3760/cma. j. issn. 1673-4408. 2021.01.002呼吸道感染是一种常见的感染性疾病，在人群中广泛存在。

The Physics of Correlated Electron Systems

The Physics of Correlated ElectronSystems在现实生活中，电子是常见的物质，我们每天都在使用电子设备，比如手机、电脑等等。

然而，当电子聚集在一起时，会出现一些奇特的物理现象，这些现象被称为“相关电子系统”的物理现象。

相关电子系统指的是多个电子相互耦合形成的量子系统，它们的性质被电子之间的相互作用所决定，因此其性质常常比单个电子更为复杂和有趣。

在“相关电子系统”中，电子之间存在着相互作用，这种相互作用可以理解为是各种微小的相互作用力在作用。

这些微小的力导致电子之间发生相互作用，从而改变电子的行为。

例如，在磁场作用下，存在一种称为“霍尔效应”的现象，这时电子会产生横向的电压，这种现象是由于不同电子在磁场中的受力不同导致的。

此外，还存在许多在相关电子系统中特有的现象，比如超导、量子霍尔效应、玻色-爱因斯坦凝聚等等。

其中，超导是相关电子系统中最具代表性的现象之一。

超导是指在特定条件下，某些物质的电阻突然消失，更为重要的是，这种物质的电导率会变得极高，达到无限大。

这种现象的发现在20世纪初期引起了许多科学家的极大兴趣，并催生了一场对新物理学的热潮。

目前，虽然超导现象的机理仍然不是十分清楚，但我们已经发现，电子之间的相互作用是超导现象的重要原因之一。

量子霍尔效应是另一个相关电子系统中的重要现象。

它是指在二维电子系统中，当磁场作用下，电子在垂直于磁场方向的电场作用下产生的电流只存在于边缘区域，而在中心区域电流为零。

这种行为进一步证明了电子之间相互作用的重要性，同时也验证了量子力学对物理学的重要贡献。

此外，在相关电子系统中，还有一种非常有趣的现象称为玻色-爱因斯坦凝聚。

在经典物理学中，玻色和爱因斯坦提出了一个关于粒子性质的假设，他们认为，具有相同自旋的一组粒子，可以处于同一个量子状态中，形成一种新的量子态，这种新态被称为玻色-爱因斯坦凝聚态。

在实验中，我们可以将冷却气体中的玻色子降温到绝对零度附近，将其束缚在一个非常小的区域内，这时玻色子会在这个区域中形成一个集体，出现一系列固有的线性和非线性的性质。

《2024年电磁辐射对神经突触可塑性影响的分子机制研究》范文

《电磁辐射对神经突触可塑性影响的分子机制研究》篇一一、引言电磁辐射已成为现代社会不可或缺的一部分，无论是移动通信、电视广播还是无线通讯技术，都在产生和传播着电磁辐射。

然而，这种无处不在的电磁辐射对人体健康的影响日益受到关注。

神经突触可塑性是神经网络学习和记忆的重要基础，因此电磁辐射对神经突触可塑性的影响尤其引人注目。

本文旨在研究电磁辐射对神经突触可塑性的影响及其分子机制。

二、电磁辐射与神经突触可塑性神经突触可塑性是指神经元之间连接强度和数量的变化，是学习和记忆的神经基础。

电磁辐射作为一种物理因素，可能通过影响神经元的电活动、神经递质的释放以及基因表达等途径，对神经突触可塑性产生影响。

三、电磁辐射对神经突触的分子机制研究（一）电磁辐射与神经元电活动研究表明，电磁辐射可以改变神经元的电活动，包括神经元的兴奋性和抑制性。

这种改变可能通过影响神经元膜上的离子通道，改变神经元的电导性，从而影响神经突触的传递和强度。

（二）电磁辐射与神经递质释放神经递质是神经突触传递信息的重要媒介。

电磁辐射可能通过影响神经递质的释放和再摄取，改变神经突触的传递效率。

例如，电磁辐射可能影响突触前膜的钙离子浓度，从而影响突触小泡的释放和神经递质的数量。

（三）电磁辐射与基因表达近年来，越来越多的研究表明，电磁辐射可以影响基因的表达。

这可能通过影响基因的转录、翻译等过程，改变相关蛋白质的合成和功能，从而影响神经突触可塑性。

例如，某些基因参与了突触的形成和维持，电磁辐射可能通过改变这些基因的表达，影响神经突触的结构和功能。

四、研究方法本研究采用分子生物学、细胞生物学和电生理学等方法，对电磁辐射对神经突触可塑性的影响进行深入研究。

具体包括：通过分子生物学技术检测相关基因的表达变化；通过细胞生物学技术观察神经元形态和突触结构的变化；通过电生理学技术记录神经元的电活动和突触传递等。

五、结论本研究发现，电磁辐射可以影响神经元电活动、神经递质释放和基因表达等过程，从而对神经突触可塑性产生影响。

用于治疗病况和疾病的反义寡聚体[发明专利]

专利名称：用于治疗病况和疾病的反义寡聚体专利类型：发明专利
发明人：伊莎贝尔·阿兹纳雷兹,韩舟
申请号：CN202210295595.2
申请日：20180824
公开号：CN114645048A
公开日：
20220621
专利内容由知识产权出版社提供
摘要：SCN1A基因中的另路剪接事件可导致非生产性mRNA转录物，其进而可导致异常的蛋白质表达，并且可靶向SCN1A基因中的另路剪接事件的治疗剂可调节Dravet综合征患者中功能性蛋白质的表达水平，并且/或者抑制异常蛋白质表达。

此类治疗剂可用来治疗由SCN1A、SCN8A或SCN5A 蛋白缺乏引起的病况。

申请人：斯托克制药公司
地址：美国马萨诸塞州
国籍：US
代理机构：北京安信方达知识产权代理有限公司
更多信息请下载全文后查看。

凝聚态物理实验第三章第二节

Related readings:
“More is Different – One more time” in “More is different” edited by Ong and Bhatt “A different universe – reinventing physics from the bottom DOWN” by Laughlin
Reference: The Nobel Prize lecture by Phillip W. Anderson
“Disordered electronic systems”, Patrick A. Lee, Rev. Mod. Phys. 57, 287 (1985) “Localization Yesterday, Today and Tomorrow” by Ramakrishnan in “More is Different”
Born 1923
According to ’s analysis of the influence of scientific research papers, Anderson is the most creative physicist in the world, followed by Steve Weinberg and Ed Witten.
extended state with mean free path l
localized state with localization length
Question:
• So far it seems the only role of disorder is to cause scattering of the Bloch waves without modifying the behavior of electrons qualitatively. What if we put in lots of impurities with strongly different impurity potential? Can the disorders cause fundamental change of the electronic

electron ptychography原理

【Electron Ptychography原理】1. Electron Ptychography介绍Electron Ptychography是一种先进的电子显微镜成像方法，它利用了电子干涉和计算机算法来实现高分辨率的样品成像。

这一技术已经广泛应用于材料科学、生物学和纳米技术领域，为研究人员提供了强大的工具来观察和分析微小尺度下的结构和特性。

2. 电子干涉原理电子干涉是指电子波在空间中叠加形成干涉图样的现象。

在电子系综的情况下，干涉效应可以通过量子力学的形式来描述。

当电子通过样品时，它们会与样品中的原子发生相互作用，导致电子波的相位和振幅发生变化。

通过测量电子波的干涉图样，可以获取有关样品的结构和性质的信息。

3. Ptychography算法Ptychography是一种通过数学算法重建样品的方法，它利用多个不同位置的电子干涉图样来提高成像分辨率。

需要将样品划分为小区域，并在每个区域使用电子束来获取干涉图样。

利用计算机算法对这些干涉图样进行处理，重建出样品的结构和性质信息。

4. Electron Ptychography的优势Electron Ptychography相比传统的电子显微镜成像方法具有很多优势。

它可以实现超高分辨率的成像，可以观察到纳米级甚至亚纳米级的结构。

它对样品的要求较低，即使是非晶态或生物样品也可以进行成像。

它还可以实现三维成像，能够观察样品的立体结构。

5. 应用领域Electron Ptychography已经在许多领域得到了广泛应用。

在材料科学领域，它可以用来研究晶体结构、界面和缺陷等。

在生物学领域，它可以用来观察生物样品的超小结构，如蛋白质、细胞器等。

在纳米技术领域，它可以用来研究纳米材料的性质和制备工艺。

6. 发展趋势随着电子显微镜技术的不断进步，Electron Ptychography也在不断发展。

未来，人们可以期待更高分辨率的成像技术的出现，以及更多领域的应用。

科学家研制出改进的磁半导体三明治材料

科学家研制出改进的磁半导体三明治材料
美国俄亥俄大学的科学家们制造了一种改进
的磁性半导体材料。这个三明治结构中间的氮化
镓和镓锰合金层几乎消灭了半导体和铁磁层间的
３结语
１）本文率先采用低温固态反应合成一系列ＢＴ１Ｚｘ３ａｉｘＯ固溶体纳米粉末（ ≤ｘ．。＿ｒ０ ≤０３该方法具）
４９０．
作者简介
冯春燕（９２，，士，事纳米介电材料的制备１８一）女硕从
与性能研究。
丁士文（９４）河北涞水人，，授，事无机合成１５一，男教从
与纳米材料方面研究。
间距增大，利于自发极化，者Ｚ的极化率大有再ｒ于Ｔ“，有利于极化，ｉ也因此相邻偶极又倾向于平行排列而形成畴结构，又会使居里点温度升高，则室温下介电常数相对降低。
（）１－８２：５１．
［ＬｕＭＬＺｏＨＬＣｅＹＲ，ｔ１Ｒｏｅ — ６］ｉ，ｈｕ，ｈｎｅａ．ｏｍＴｍ
ｐｒｔｒＳｌ－ｏｉＲａｔｎｒｐｒｔｎｆＩｏｅａｕｅｏｉｓｌｄｄｅｃｉＰｅａａｉｏｒｎｏｏ
维普资讯
第５期
２００６年ｌ０月
纳
米
科
技

相互作用因子

相互作用因子
相互作用因子（Interacting Factor）是指在生物学、医学和物理学等领域中，两个或多个因素之间相互影响、相互作用，从而产生某种效应的因素。

这些因素可以是生物分子、细胞、组织、器官、个体、种群或生态系统等，它们之间的相互作用可以导致各种复杂的生物学和物理学现象。

在生物学中，相互作用因子可以指基因与基因、基因与蛋白质、蛋白质与蛋白质之间的相互作用，这些相互作用可以调节细胞代谢、信号转导、基因表达等过程。

在医学中，相互作用因子可以指药物与药物、药物与受体、药物与酶之间的相互作用，这些相互作用可以影响药物的疗效和安全性。

在物理学中，相互作用因子可以指粒子与粒子、粒子与场之间的相互作用，这些相互作用可以导致各种物理现象，如电磁场、引力场、量子纠缠等。

研究相互作用因子的意义在于深入了解各种生物学和物理学现象的本质和机制，为疾病的诊断和治疗提供理论支持和实践指导。

例如，研究肿瘤细胞中基因与基因的相互作用可以帮助我们更好地理解肿瘤的发生和发展机制，从而开发更有效的肿瘤治疗药物。

研究药物与药物的相互作用可以帮助医生更好地掌握药物配伍的原则和方法，避免药物不良反应的发生。

研究粒子与粒子的相互作用可以帮助我们更好地理解宇宙的起源和演化，探索新的物理规律和现象。

总之，相互作用因子是生物学、医学和物理学等领域中非常重要的概念，它可以帮助我们更好地理解各种现象的本质和机制，为未来的科学研究和技术创新提供基础和支持。

纳米材料专业英语

第一章晶体结构缺陷 defect, imperfection 点缺陷 point defect线缺陷 line defect, dislocation 面缺陷 interface defect体缺陷 volume defect 位错排列 dislocation arrangement位错线 dislocation line 刃位错 edge dislocation螺位错 screw dislocation 混合位错 mixed dislocation晶界 grain boundaries 大角度晶界 high-angle grain boundaries小角度晶界 tilt boundary, 孪晶界 twin boundaries位错阵列 dislocation array 位错气团 dislocation atmosphere位错轴 dislocation axis 位错胞 dislocation cell位错爬移 dislocation climb 位错聚结 dislocation coalescence位错滑移 dislocation slip 位错核心能量 dislocation core energy位错裂纹 dislocation crack 位错阻尼 dislocation damping位错密度 dislocation density 原子错位 substitution of a wrong atom 间隙原子 interstitial atom 晶格空位 vacant lattice sites间隙位置 interstitial sites 杂质 impurities弗伦克尔缺陷 Frenkel disorder 肖脱基缺陷 Schottky disorder主晶相 the host lattice 错位原子 misplaced atoms缔合中心 Associated Centers. 自由电子 Free Electrons电子空穴 Electron Holes 伯格斯矢量 Burgers克罗各-明克符号 Kroger Vink notation 中性原子 neutral atom原子质量单位 Atomic mass unit (amu) 原子数 Atomic number原子量 Atomic weight 波尔原子模型 Bohr atomic model键能 Bonding energy 库仑力 Coulombic force共价键 Covalent bond 分子的构型 molecular configuration电子构型 electronic configuration 负电的 Electronegative正电的 Electropositive 基态 Ground state氢键 Hydrogen bond 离子键 Ionic bond同位素 Isotope 金属键 Metallic bond摩尔 Mole 分子 Molecule泡利不相容原理 Pauli exclusion principle 元素周期表 Periodic table原子 atom 分子 molecule分子量 molecule weight 极性分子 Polar molecule量子数 quantum number 价电子 valence electron范德华键 van der waals bond 电子轨道 electron orbitals点群 point group 对称要素 symmetry elements各向异性 anisotropy 原子堆积因数 atomic packing factor(APF)体心立方结构 body-centered cubic (BCC) 面心立方结构 face-centered cubic (FCC)布拉格定律bragg’s law配位数 coordination number晶体结构 crystal structure 晶系 crystal system晶体的 crystalline 衍射 diffraction中子衍射 neutron diffraction 电子衍射 electron diffraction晶界 grain boundary 六方密堆积 hexagonal close-packed (HCP)鲍林规则Pauling’s rules NaCl型结构 NaCl－type structureCsCl型结构 Caesium Chloride structure 闪锌矿型结构 Blende-type structure 纤锌矿型结构 Wurtzite structure 金红石型结构 Rutile structure萤石型结构 Fluorite structure 钙钛矿型结构 Perovskite-type structure尖晶石型结构 Spinel-type structure 硅酸盐结构 Structure of silicates岛状结构 Island structure 链状结构 Chain structure层状结构 Layer structure 架状结构 Framework structure滑石 talc 叶蜡石 pyrophyllite高岭石 kaolinite 石英 quartz长石 feldspar 美橄榄石 forsterite各向同性的 isotropic 各向异性的 anisotropy晶格 lattice 晶格参数 lattice parameters密勒指数 miller indices 非结晶的 noncrystalline多晶的 polycrystalline 多晶形 polymorphism单晶 single crystal 晶胞 unit cell电位 electron states (化合)价 valence电子 electrons 共价键 covalent bonding金属键 metallic bonding 离子键 Ionic bonding极性分子 polar molecules 原子面密度 atomic planar density衍射角 diffraction angle 合金 alloy 配位数 coordination number粒度,晶粒大小 grain size 显微结构 microstructure显微照相 photomicrograph 扫描电子显微镜 scanning electron microscope (SEM)透射电子显微镜 Transmission electron microscope (TEM)重量百分数 weight percent 四方的 tetragonal 单斜的 monoclinic第二章晶体结构缺陷-固溶体固溶度 solid solubility 间隙固溶体 interstitial solid solution金属间化合物 intermetallics 转熔型固溶体 peritectic solid solution无序固溶体 disordered solid solution取代型固溶体 Substitutional solid solutions非化学计量化合物 Nonstoichiometric compound第三章熔体结构熔体结构 structure of melt 过冷液体 supercooling melt玻璃态 vitreous state 软化温度 softening temperature粘度 viscosity 表面张力 Surface tension介稳态过渡相 metastable phase 组织 constitution淬火 quenching 退火的 softened玻璃分相 phase separation in glasses 体积收缩 volume shrinkage第四章固体的表面与界面表面 surface 界面 interface 惯习面 habit plane同相界面 homophase boundary 异相界面 heterophase boundary晶界 grain boundary 表面能 surface energy小角度晶界 low angle grain boundary 大角度晶界 high angle grain boundary 共格孪晶界 coherent twin boundary 晶界迁移 grain boundary migration错配度 mismatch 驰豫 relaxation重构 reconstuction 表面吸附 surface adsorption表面能 surface energy 倾转晶界 titlt grain boundary扭转晶界 twist grain boundary 倒易密度 reciprocal density共格界面 coherent boundary 半共格界面 semi-coherent boundary非共格界面 noncoherent boundary 界面能 interfacial free energy应变能 strain energy 晶体学取向关系 crystallographic orientation 第五章相图相图 phase diagrams 相 phase 组分 component 组元 compoonent 相律 Phase rule 投影图 Projection drawing浓度三角形 Concentration triangle 冷却曲线 Cooling curve成分 composition 自由度 freedom相平衡 phase equilibrium 化学势 chemical potential热力学 thermodynamics 相律 phase rule吉布斯相律 Gibbs phase rule 自由能 free energy吉布斯自由能 Gibbs free energy 吉布斯混合能 Gibbs energy of mixing 吉布斯熵 Gibbs entropy 吉布斯函数 Gibbs function热力学函数 thermodynamics function 热分析 thermal analysis过冷 supercooling 过冷度 degree of supercooling杠杆定律 lever rule 相界 phase boundary相界线 phase boundary line 相界交联 phase boundary crosslinking共轭线 conjugate lines 相界有限交联 phase boundary crosslinking相界反应 phase boundary reaction 相变 phase change相组成 phase composition 共格相 phase-coherent金相相组织 phase constentuent 相衬 phase contrast相衬显微镜 phase contrast microscope 相衬显微术 phase contrast microscopy 相分布 phase distribution 相平衡常数 phase equilibrium constant相平衡图 phase equilibrium diagram 相变滞后 phase transition lag相分离 phase segregation 相序 phase order相稳定性 phase stability 相态 phase state相稳定区 phase stabile range 相变温度 phase transition temperature相变压力phase transition pressure 同质多晶转变polymorphic transformation同素异晶转变allotropic transformation 相平衡条件phase equilibrium conditions显微结构 microstructures 低共熔体 eutectoid不混溶性 immiscibility第六章扩散下坡扩散 Downhill diffusion 互扩散系数 Mutual diffusion渗碳剂 carburizing 浓度梯度 concentration gradient浓度分布曲线 concentration profile 扩散流量 diffusion flux驱动力 driving force 间隙扩散 interstitial diffusion自扩散 self-diffusion 表面扩散 surface diffusion空位扩散 vacancy diffusion 扩散偶 diffusion couple扩散方程 diffusion equation 扩散机理 diffusion mechanism扩散特性 diffusion property 无规行走 Random walk达肯方程 Dark equation 柯肯达尔效应 Kirkendall equation本征热缺陷 Intrinsic thermal defect 本征扩散系数 Intrinsic diffusion coefficient 离子电导率 Ion-conductivity 空位机制 Vacancy concentration第七章相变过冷 supercooling 过冷度 degree of supercooling晶核 nucleus 形核 nucleation形核功 nucleation energy 晶体长大 crystal growth均匀形核 homogeneous nucleation 非均匀形核 heterogeneous nucleation形核率 nucleation rate 长大速率 growth rate热力学函数 thermodynamics function临界晶核 critical nucleus 临界晶核半径 critical nucleus radius枝晶偏析 dendritic segregation 局部平衡 localized equilibrium平衡分配系数 equilibrium distributioncoefficient 有效分配系数 effective distribution coefficient成分过冷 constitutional supercooling 引领（领现相） leading phase共晶组织 eutectic structure 层状共晶体 lamellar eutectic伪共晶 pseudoeutectic 离异共晶 divorsed eutectic表面等轴晶区 chill zone 柱状晶区 columnar zone中心等轴晶区 equiaxed crystal zone 定向凝固 unidirectional solidification 急冷技术 splatcooling 区域提纯 zone refining单晶提拉法 Czochralski method 晶界形核 boundary nucleation位错形核 dislocation nucleation 晶核长大 nuclei growth斯宾那多分解spinodal decomposition 有序无序转变disordered-order transition马氏体相变 martensite phase transformation 马氏体 martensite第八、九章固相反应和烧结固相反应 solid state reaction 烧结 sintering烧成 fire 合金 alloy再结晶 Recrystallization 二次再结晶 Secondary recrystallization成核 nucleation 结晶 crystallization子晶，雏晶 matted crystal 耔晶取向 seed orientation异质核化heterogeneous nucleation 均匀化热处理homogenization heat treatment铁碳合金 iron-carbon alloy 渗碳体 cementite铁素体 ferrite 奥氏体 austenite共晶反应 eutectic reaction 固溶处理 solution heat treatment。

介电陶瓷／ＮｉＺｎ铁氧体互扩散行为的第一性原理研究

第３８卷　第４期２０２３年１２月西　南　科　技　大　学　学　报ＪｏｕｒｎａｌｏｆＳｏｕｔｈｗｅｓｔＵｎｉｖｅｒｓｉｔｙｏｆＳｃｉｅｎｃｅａｎｄＴｅｃｈｎｏｌｏｇｙＶｏｌ．３８Ｎｏ．４Ｄｅｃ．２０２３ＤＯＩ：１０．２００３６／ｊ．ｃｎｋｉ．１６７１８７５５．２０２３．０４．００７收稿日期：２０２３－０２－２７；修回日期：２０２３－０５－１６作者简介：第一作者，张凯（１９９７—），男，硕士研究生；通信作者，毕鹏（１９８５—），博士，讲师，研究方向为计算材料学，Ｅｍａｉｌ：ｂｉｐｅｎｇ０１０＠ｓｗｕｓｔ．ｅｄｕ．ｃｎ介电陶瓷／ＮｉＺｎ铁氧体互扩散行为的第一性原理研究张　凯１　郭子康１　刘振涛１　毕　鹏２（１．西南科技大学材料与化学学院　四川绵阳　６２１０１０；２．西南科技大学数理学院　四川绵阳　６２１０１０）摘要：针对介电陶瓷／ＮｉＺｎ铁氧体异质复合材料的低温共烧陶瓷体系，建立掺杂结构模型，采用基于密度泛函理论的第一性原理计算并结合ＣＩ－ＮＥＢ方法研究阳离子互扩散机制。

结果表明：铁氧体中的Ｎｉ，Ｚｎ，Ｆｅ离子主要取代介电陶瓷ＭｇＴｉＯ３和ＣａＴｉＯ３体系中的Ｔｉ位，迁移势垒１．０～５．５ｅＶ；对于介电陶瓷Ｍｇ和Ｃａ倾向于占据ＮｉＺｎＦｅ４Ｏ８中Ｚｎ位点，Ｔｉ则倾向于取代Ｆｅ位，迁移势垒０．６～１．０ｅＶ。

对于该材料体系，典型共烧工艺条件下Ｃａ，Ｍｇ扩散进入ＮｉＺｎ铁氧体距离４００～１０００μｍ。

关键词：介电陶瓷／铁氧体共烧体系　掺杂　互扩散　第一性原理中图分类号：ＴＱ１７４．１文献标志码：Ａ文章编号：１６７１－８７５５（２０２３）０４－００４５－０９ＦｉｒｓｔｐｒｉｎｃｉｐｌｅｓＳｔｕｄｙｏｆＤｉｆｆｕｓｉｏｎＢｅｈａｖｉｏｒｂｅｔｗｅｅｎＤｉｅｌｅｃｔｒｉｃＣｅｒａｍｉｃｓａｎｄＮｉＺｎｆｅｒｒｉｔｅＺＨＡＮＧＫａｉ１，ＧＵＯＺｉｋａｎｇ１，ＬＩＵＺｈｅｎｔａｏ１，ＢＩＰｅｎｇ２（１．ＳｃｈｏｏｌｏｆＭａｔｅｒｉａｌｓａｎｄＣｈｅｍｉｓｔｒｙ，ＳｏｕｔｈｗｅｓｔＵｎｉｖｅｒｓｉｔｙｏｆＳｃｉｅｎｃｅａｎｄＴｅｃｈｎｏｌｏｇｙ，Ｍｉａｎｙａｎｇ６２１０１０，Ｓｉｃｈｕａｎ，Ｃｈｉｎａ；２．ＳｃｈｏｏｌｏｆＳｃｉｅｎｃｅ，ＳｏｕｔｈｗｅｓｔＵｎｉｖｅｒｓｉｔｙｏｆＳｃｉｅｎｃｅａｎｄＴｅｃｈｎｏｌｏｇｙ，Ｍｉａｎｙａｎｇ６２１０１０，Ｓｉｃｈｕａｎ，Ｃｈｉｎａ）Ａｂｓｔｒａｃｔ：ＦｏｒｔｈｅｌｏｗｔｅｍｐｅｒａｔｕｒｅｃｏｆｉｒｅｄｃｅｒａｍｉｃｓｙｓｔｅｍｏｆｔｈｅｄｉｅｌｅｃｔｒｉｃｃｅｒａｍｉｃａｎｄＮｉＺｎ－ｆｅｒｒｉｔｅ，ｔｈｅｄｏｐｉｎｇｍｏｄｅｌｉｓｅｓｔａｂｌｉｓｈｅｄ，ａｎｄｔｈｅｃａｔｉｏｎｍｕｔｕａｌｄｉｆｆｕｓｉｏｎｍｅｃｈａｎｉｓｍｉｓｓｔｕｄｉｅｄｂｙｕｓｉｎｇｔｈｅｆｉｒｓｔｐｒｉｎｃｉｐｌｅｓｃａｌｃｕｌａｔｉｏｎｂａｓｅｄｏｎｔｈｅＤＦＴａｎｄＣＩ－ＮＥＢｍｅｔｈｏｄ．ＴｈｅｒｅｓｕｌｔｓｓｈｏｗｔｈａｔＮｉ，ＺｎａｎｄＦｅｉｎｆｅｒｒｉｔｅｍａｉｎｌｙｒｅｐｌａｃｅｔｈｅＴｉｉｎｔｈｅｄｉｅｌｅｃｔｒｉｃｃｅｒａｍｉｃＭｇＴｉＯ３ａｎｄＣａＴｉＯ３，ａｎｄｔｈｅｍｉｇｒａｔｉｏｎｂａｒｒｉｅｒｉｓ１．０－５．５ｅＶ；Ｆｏｒｄｉｅｌｅｃｔｒｉｃｃｅｒａｍｉｃｓ，ＭｇａｎｄＣａｔｅｎｄｔｏｏｃｃｕｐｙｔｈｅＺｎｓｉｔｅｉｎＮｉＺｎＦｅ４Ｏ８，ｗｈｉｌｅＴｉｔｅｎｄｓｔｏｒｅｐｌａｃｅｔｈｅＦｅｓｉｔｅ，ａｎｄｔｈｅｍｉｇｒａｔｉｏｎｂａｒｒｉｅｒｉｓ０．６－１．０ｅＶ．Ｆｏｒｔｈｉｓｍａｔｅｒｉａｌｓｙｓｔｅｍ，ｕｎｄｅｒｔｙｐｉｃａｌｃｏｆｉｒｉｎｇｐｒｏｃｅｓｓｃｏｎｄｉｔｉｏｎｓ，ＣａａｎｄＭｇｄｉｆｆｕｓｅｉｎｔｏＮｉＺｎｆｅｒｒｉｔｅａｔａｄｉｓｔａｎｃｅ４００－１０００μｍ．Ｋｅｙｗｏｒｄｓ：Ｄｉｅｌｅｃｔｒｉｃｃｅｒａｍｉｃ／ｆｅｒｒｉｔｅｃｏｆｉｒｉｎｇｓｙｓｔｅｍ；Ｄｏｐｉｎｇ；Ｍｕｔｕａｌｄｉｆｆｕｓｉｏｎ；Ｆｉｒｓｔｐｒｉｎｃｉｐｌｅｓ伴随５Ｇ时代的到来，电子器件在片式小型化的同时朝着高性能、多功能、高可靠的方向发展。

细胞凋亡的线粒体途径

Mitochondrial Pathways in physiological cell death
If mitochondria are pivotal in controlling cell life and death, then how do these organelles kill? At least three general mechanisms are known, and their effects may be interrelated, including (i) disruption of electron transport, oxidative phosphorylation, and adenosine triphosphate (ATP) production; (ii) release of proteins that trigger activation of caspase family proteases; and (iii) alteration of cellular reduction-oxidation (redox) potential
disruptionofelectrontransportandenergymetabolism线粒体跨膜电位的耗散与细胞凋亡有密切关系近年来陆续有报道说明线粒体跨膜电位的耗散早于核酸酶的激活也早于磷酯酰丝氨酸暴露于细胞表面
细胞凋亡的线粒体途径
蒋舜媛董霞程在全
2002-12-17
细胞死亡损伤性死亡 Nhomakorabea生化特征:
染色质降解, 核小体间连接DNA部位被降解,产生寡聚核小体DNA片段,即180-200DP 整数倍的不同长度的DNA片断.
Fig.1. Schematic summary of biochemical mechanisms of apoptosis.

多通道碳阴极活化过一硫酸盐降解水中有机物的性能

大连理工大学硕士学位论文摘要活化的过硫酸盐氧化，作为一种新兴的高级氧化技术，是一种矿化难降解有毒污染物的有效方法。

在众多的活化方法中，过硫酸盐通过接受电子完成的电化学活化，具有容易操控和环境友好的特点，被认为是一种有前景的活化技术。

但在电化学活化的过程中，由于静电斥力阻碍了过硫酸盐阴离子和阴极之间的接触，导致过硫酸盐低的分解率和随后低的自由基的产生量，从而使污染物的降解效果变差。

针对此问题，本文使用天然木材衍生的碳化木（CW）制备了具有多通道的流通式阴极（FTC），通过将过一硫酸盐（PMS）阴离子限制在阴极的微通道中，能够显著地强化其与阴极的碰撞与接触，提高电化学活化的效率并增强对污染物的降解。

主要的研究成果如下：（1）通过天然松木的一步碳化制备并组装了具有丰富的介孔，良好的导电性，较高的机械强度，大量有序的微通道以及对PMS有良好的电催化活性的FTC。

以苯酚为目标污染物，探究了不同的反应条件（PMS浓度、电流密度和停留时间）对FTC电活化PMS降解苯酚性能的影响。

结果表明，在苯酚进水浓度为20 mg/L, 进水TOC=18 mg/L，进水PMS浓度为6.51 mM，背景Na2SO4为0.05 M，电流密度为2.75 mA/cm2，进水pH 2.87，停留时间10 min以及常温的条件下，通过FTC电活化PMS，PMS的分解率达到了71.9%。

苯酚和TOC的去除率分别达到了97.9%和39.6%。

EPR实验结果表明，在FTC电活化PMS的过程中，产生了大量的·OH和SO4•-。

同时，自由基淬灭实验也表明，·OH和SO4•-均参与了对苯酚的降解，且·OH对降解的贡献更大。

此外，五次循环实验的结果证明了本研究组装的FTC具有很好的稳定性。

（2）通过封闭CW的微通道，获得了流过式阴极（FBC）。

在相同的优化条件下，详细对比了在FTC中和FBC上的PMS的分解、自由基的产量以及电活化PMS降解三种酚类有机物（苯酚、双酚A和4-氯苯酚）的性能。

C反应蛋白检测与临床诊断思路

(a) Negatively stained electron micrograph showing the typical pentameric disc-like structure face-on and side-on (arrows).
(b) Ribbon diagram of the crystal structure, showing the lectin fold and the two calcium atoms (spheres) in the ligand-binding site of each protomer .
CRP--急性时相反应的一个极灵敏的指标
各种组织损伤
急性心肌梗死
栓塞
创伤
感染
炎症
P
外科手术肿瘤浸润
R
风湿病
C
炎症：
6~12h可检测升高
18~24h达到高峰
可达正常水平的数十至数百倍，甚至2000 倍。
CRP
是炎症或组织损
伤的非特异性标志物
CRP--急性时相反应的一个极灵敏的指标
半衰期短，5～7h，达峰值后，以每天近50%的速度迅速趋向正常，随着病情的缓解，病变消退、组织结构和功能的恢复，其浓度可迅速降至正常水平，与病情变化同步且与病情的严重程度具有相关性。
CRP变化的临床思路
9.良恶性胸腔积液的鉴别诊断
炎性胸腔积液CRP水平＞结核性胸腔积液 CRP水平＞恶性胸腔积液CRP水平
血、胸液CRP＜ 5.0mg/L见于癌性胸腔积液不支持结核性胸腔积液
结核性胸液患者血/胸液CRP＜ 1 癌性胸液患者血/胸液CRP基本上≥1
CRP变化的临床思路
10.免疫性疾病的判断

中国诺奖级别新科技—量子反常霍尔效应英语

中国诺奖级别新科技—量子反常霍尔效应英语全文共6篇示例，供读者参考篇1The Magical World of Quantum PhysicsHave you ever heard of something called quantum physics? It's a fancy word that describes the weird and wonderful world of tiny, tiny particles called atoms and electrons. These particles are so small that they behave in ways that seem almost magical!One of the most important discoveries in quantum physics is something called the Quantum Anomalous Hall Effect. It's a mouthful, I know, but let me try to explain it to you in a way that's easy to understand.Imagine a road, but instead of cars driving on it, you have electrons zipping along. Now, normally, these electrons would bump into each other and get all mixed up, just like cars in a traffic jam. But with the Quantum Anomalous Hall Effect, something special happens.Picture a big, strong police officer standing in the middle of the road. This police officer has a magical power – he can makeall the electrons go in the same direction, without any bumping or mixing up! It's like he's directing traffic, but for tiny particles instead of cars.Now, you might be wondering, "Why is this so important?" Well, let me tell you! Having all the electrons moving in the same direction without any resistance means that we can send information and electricity much more efficiently. It's like having a super-smooth highway for the electrons to travel on, without any potholes or roadblocks.This discovery was made by a team of brilliant Chinese scientists, and it's so important that they might even win a Nobel Prize for it! The Nobel Prize is like the Olympic gold medal of science – it's the highest honor a scientist can receive.But the Quantum Anomalous Hall Effect isn't just about winning awards; it has the potential to change the world! With this technology, we could create faster and more powerful computers, better ways to store and transfer information, and even new types of energy篇2China's Super Cool New Science Discovery - The Quantum Anomalous Hall EffectHey there, kids! Have you ever heard of something called the "Quantum Anomalous Hall Effect"? It's a really cool andmind-boggling scientific discovery that scientists in China have recently made. Get ready to have your mind blown!Imagine a world where electricity flows without any resistance, like a river without any rocks or obstacles in its way. That's basically what the Quantum Anomalous Hall Effect is all about! It's a phenomenon where electrons (the tiny particles that carry electricity) can flow through a material without any resistance or energy loss. Isn't that amazing?Now, you might be wondering, "Why is this such a big deal?" Well, let me tell you! In our regular everyday world, when electricity flows through materials like wires or circuits, there's always some resistance. This resistance causes energy to be lost as heat, which is why your phone or computer gets warm when you use them for a long time.But with the Quantum Anomalous Hall Effect, the electrons can flow without any resistance at all! It's like they're gliding effortlessly through the material, without any obstacles or bumps in their way. This means that we could potentially have electronic devices and circuits that don't generate any heat or waste any energy. How cool is that?The scientists in China who discovered this effect were studying a special kind of material called a "topological insulator." These materials are like a secret passageway for electrons, allowing them to flow along the surface without any resistance, while preventing them from passing through the inside.Imagine a river flowing on top of a giant sheet of ice. The water can flow freely on the surface, but it can't pass through the solid ice underneath. That's kind of how these topological insulators work, except with electrons instead of water.The Quantum Anomalous Hall Effect happens when these topological insulators are exposed to a powerful magnetic field. This magnetic field creates a special condition where the electrons can flow along the surface without any resistance at all, even at room temperature!Now, you might be thinking, "That's all well and good, but what does this mean for me?" Well, this discovery could lead to some pretty amazing things! Imagine having computers and electronic devices that never overheat or waste energy. You could play video games or watch movies for hours and hours without your devices getting hot or draining their batteries.But that's not all! The Quantum Anomalous Hall Effect could also lead to new and improved ways of generating, storing, and transmitting energy. We could have more efficient solar panels, better batteries, and even a way to transmit electricity over long distances without any energy loss.Scientists all around the world are really excited about this discovery because it opens up a whole new world of possibilities for technology and innovation. Who knows what kind of cool gadgets and devices we might see in the future thanks to the Quantum Anomalous Hall Effect?So, there you have it, kids! The Quantum Anomalous Hall Effect is a super cool and groundbreaking scientific discovery that could change the way we think about electronics, energy, and technology. It's like something straight out of a science fiction movie, but it's real and happening right here in China!Who knows, maybe one day you'll grow up to be a scientist and help us unlock even more amazing secrets of the quantum world. Until then, keep learning, keep exploring, and keep being curious about the incredible wonders of science!篇3The Wonderful World of Quantum Physics: A Journey into the Quantum Anomalous Hall EffectHave you ever heard of something called quantum physics? It's a fascinating field that explores the strange and mysterious world of tiny particles called atoms and even smaller things called subatomic particles. Imagine a world where the rules we're used to in our everyday lives don't quite apply! That's the world of quantum physics, and it's full of mind-boggling discoveries and incredible phenomena.One of the most exciting and recent breakthroughs in quantum physics comes from a team of brilliant Chinese scientists. They've discovered something called the Quantum Anomalous Hall Effect, and it's like a magic trick that could change the way we think about technology!Let me start by telling you a bit about electricity. You know how when you turn on a light switch, the bulb lights up? That's because electricity is flowing through the wires and into the bulb. But did you know that electricity is actually made up of tiny particles called electrons? These electrons flow through materials like metals and give us the electricity we use every day.Now, imagine if we could control the flow of these electrons in a very precise way, like directing them to move in a specificdirection without any external forces like magnets or electric fields. That's exactly what the Quantum Anomalous Hall Effect allows us to do!You see, in most materials, electrons can move in any direction, like a group of kids running around a playground. But in materials that exhibit the Quantum Anomalous Hall Effect, the electrons are forced to move in a specific direction, like a group of kids all running in a straight line without any adults telling them where to go!This might not seem like a big deal, but it's actually a huge deal in the world of quantum physics and technology. By controlling the flow of electrons so precisely, we can create incredibly efficient electronic devices and even build powerful quantum computers that can solve problems much faster than regular computers.The Chinese scientists who discovered the Quantum Anomalous Hall Effect used a special material called a topological insulator. This material is like a magician's hat – it looks ordinary on the outside, but it has some really weird and wonderful properties on the inside.Inside a topological insulator, the electrons behave in a very strange way. They can move freely on the surface of the material, but they can't move through the inside. It's like having篇4The Coolest New Science from China: Quantum Anomalous Hall EffectHey kids! Have you ever heard of something called the Quantum Anomalous Hall Effect? It's one of the most amazing new scientific discoveries to come out of China. And get this - some scientists think it could lead to a Nobel Prize! How cool is that?I know, I know, the name sounds kind of weird and complicated. But trust me, once you understand what it is, you'll think it's just as awesome as I do. It's all about controlling the movement of tiny, tiny particles called electrons using quantum physics and powerful magnetic fields.What's Quantum Physics?Before we dive into the Anomalous Hall Effect itself, we need to talk about quantum physics for a second. Quantum physics is sort of like the secret rules that govern how the smallest things inthe universe behave - things too tiny for us to even see with our eyes!You know how sometimes grown-ups say things like "You can't be in two places at once"? Well, in the quantum world, particles actually can be in multiple places at the same time! They behave in ways that just seem totally bizarre and counterintuitive to us. That's quantum physics for you.And get this - not only can quantum particles be in multiple places at once, but they also spin around like tops! Electrons, which are one type of quantum particle, have this crazy quantum spin that makes them act sort of like tiny magnets. Mind-blowing, right?The Weirder Than Weird Hall EffectOkay, so now that we've covered some quantum basics, we can talk about the Hall Effect. The regular old Hall Effect was discovered way back in 1879 by this dude named Edwin Hall (hence the name).Here's how it works: if you take a metal and apply a magnetic field to it while also running an electrical current through it, the magnetic field will actually deflect the flow of electrons in the metal to one side. Weird, huh?Scientists use the Hall Effect in all kinds of handy devices like sensors, computer chips, and even machines that can shoot out a deadly beam of radiation (just kidding on that last one...I think). But the regular Hall Effect has one big downside - it only works at incredibly cold temperatures near absolute zero. Not very practical!The Anomalous Hall EffectThis is where the new Quantum Anomalous Hall Effect discovered by scientists in China comes into play. They found a way to get the same cool electron-deflecting properties of the Hall Effect, but at much higher, more realistic temperatures. And they did it using some crazy quantum physics tricks.You see, the researchers used special materials called topological insulators that have insulating interiors but highly conductive surfaces. By sandwiching these topological insulators between two layers of magnets, they were able to produce a strange quantum phenomenon.Electrons on the surface of the materials started moving in one direction without any external energy needed to keep them going! It's like they created a perpetual motion machine for electrons on a quantum scale. The spinning quantum particlesget deflected by the magnetic layers and start flowing in weird looping patterns without any resistance.Why It's So AwesomeSo why is this Quantum Anomalous Hall Effect such a big deal? A few reasons:It could lead to way more efficient electronics that don't waste energy through heat and resistance like current devices do. Just imagine a computer chip that works with virtually no power at all!The effect allows for extremely precise control over the movement of electrons, which could unlock all kinds of crazy quantum computing applications we can barely even imagine yet.It gives scientists a totally new window into understanding the bizarre quantum realm and the funky behavior of particles at that scale.The materials used are relatively inexpensive and common compared to other cutting-edge quantum materials. So this isn't just a cool novelty - it could actually be commercialized one day.Some Science Celebrities Think It's Nobel-WorthyLots of big-shot scientists around the world are going gaga over this Quantum Anomalous Hall Effect discovered by the researchers in China. A few have even said they think it deserves a Nobel Prize!Now, as cool as that would be, we have to remember that not everyone agrees it's Nobel-level just yet. Science moves slow and there's always a ton of debate over what discoveries are truly groundbreaking enough to earn that high honor.But one thing's for sure - this effect is yet another example of how China is becoming a global powerhouse when it comes to cutting-edge physics and scientific research. Those Chinese scientists are really giving their counterparts in the US, Europe, and elsewhere a run for their money!The Future is QuantumWhether the Quantum Anomalous Hall Effect leads to a Nobel or not, one thing is certain - we're entering an age where quantum physics is going to transform technology in ways we can barely fathom right now.From quantum computers that could solve problems millions of times faster than today's machines, to quantum sensors that could detect even the faintest subatomic particles,to quantum encryption that would make data unhackable, this strange realm of quantum physics is going to change everything.So pay attention, kids! Quantum physics may seem like some weird, headache-inducing mumbo-jumbo now. But understanding these bizarre quantum phenomena could be the key to unlocking all the super-cool technologies of the future. Who knows, maybe one of you reading this could even grow up to be a famous quantum physicist yourselves!Either way, keep your eyes peeled for more wild quantum discoveries emerging from China and other science hotspots around the globe. The quantum revolution is coming, and based on amazing feats like the Anomalous Hall Effect, it's going to be one heckuva ride!篇5Whoa, Dudes! You'll Never Believe the Insanely Cool Quantum Tech from China!Hey there, kids! Get ready to have your minds totally blown by the most awesome scientific discovery ever - the quantum anomalous Hall effect! I know, I know, it sounds like a bunch of big, boring words, but trust me, this stuff is straight-upmind-blowing.First things first, let's talk about what "quantum" means. You know how everything in the universe is made up of tiny, tiny particles, right? Well, quantum is all about studying those teeny-weeny particles and how they behave. It's like a whole secret world that's too small for us to see with our eyes, but scientists can still figure it out with their mega-smart brains and super-powerful microscopes.Now, let's move on to the "anomalous Hall effect" part. Imagine you're a little electron (that's one of those tiny particles I was telling you about) and you're trying to cross a busy street. But instead of just going straight across, you get pushed to the side by some invisible force. That's kind of what the Hall effect is all about - electrons getting pushed sideways instead of going straight.But here's where it gets really cool: the "anomalous" part means that these electrons are getting pushed sideways even when there's no magnetic field around! Normally, you'd need a powerful magnet to make electrons move like that, but with this new quantum technology, they're doing it all by themselves. It's like they've got their own secret superpowers or something!Now, you might be wondering, "Why should I care about some silly electrons moving around?" Well, let me tell you, thisdiscovery is a huge deal! You see, scientists have been trying to figure out how to control the flow of electrons for ages. It's kind of like trying to herd a bunch of rowdy puppies - those little guys just want to go wherever they want!But with this new quantum anomalous Hall effect, scientists in China have finally cracked the code. They've found a way to make electrons move in a specific direction without any external forces. That means they can control the flow of electricity like never before!Imagine having a computer that never overheats, or a smartphone that never runs out of battery. With this new technology, we could create super-efficient electronic devices that waste way less energy. It's like having a magical power switch that can turn on and off the flow of electrons with just a flick of a wrist!And that's not even the coolest part! You know how sometimes your electronics get all glitchy and stop working properly? Well, with this quantum tech, those problems could be a thing of the past. See, the anomalous Hall effect happens in special materials called "topological insulators," which are like super-highways for electrons. No matter how many twists andturns they take, those little guys can't get lost or stuck in traffic jams.It's like having a navigation system that's so good, you could close your eyes and still end up at the right destination every single time. Pretty neat, huh?But wait, there's more! Scientists are also exploring the possibility of using this new technology for quantum computing. Now, I know you're probably thinking, "What the heck is quantum computing?" Well, let me break it down for you.You know how regular computers use ones and zeros to process information, right? Well, quantum computers use something called "qubits," which can exist as both one and zero at the same time. It's like having a coin that's heads and tails at the same exact moment - totally mind-boggling, I know!With this quantum anomalous Hall effect, scientists might be able to create super-stable qubits that can perform insanely complex calculations in the blink of an eye. We're talking about solving problems that would take regular computers millions of years to figure out. Imagine being able to predict the weather with 100% accuracy, or finding the cure for every disease known to humankind!So, what do you say, kids? Are you as pumped about this as I am? I know it might seem like a lot of mumbo-jumbo right now, but trust me, this is the kind of stuff that's going to change the world as we know it. Who knows, maybe one day you'll be the one working on the next big quantum breakthrough!In the meantime, keep your eyes peeled for more news about this amazing discovery from China. And remember, even though science can be super complicated sometimes, it's always worth paying attention to. After all, you never know when the next mind-blowing quantum secret might be revealed!篇6Title: A Magical Discovery in the World of Tiny Particles!Have you ever heard of something called the "Quantum Anomalous Hall Effect"? It might sound like a tongue twister, but it's actually a super cool new technology that was recently discovered by scientists in China!Imagine a world where everything is made up of tiny, tiny particles called atoms. These atoms are so small that you can't see them with your bare eyes, but they're the building blocks that make up everything around us – from the chair you're sitting on to the air you breathe.Now, these atoms can do some pretty amazing things when they're arranged in certain ways. Scientists have found that if they create special materials where the atoms are arranged just right, they can make something called an "electrical current" flow through the material without any resistance!You might be wondering, "What's so special about that?" Well, let me explain! Usually, when electricity flows through a material like a metal wire, it faces something called "resistance." This resistance makes it harder for the electricity to flow, kind of like trying to run through a thick forest – it's tough and you get slowed down.But with this new Quantum Anomalous Hall Effect, the electricity can flow through the special material without any resistance at all! It's like having a wide-open road with no obstacles, allowing the electricity to zoom through without any trouble.So, how does this magical effect work? It all comes down to the behavior of those tiny atoms and the way they interact with each other. You see, in these special materials, the atoms are arranged in a way that creates a kind of "force field" that protects the flow of electricity from any resistance.Imagine you're a tiny particle of electricity, and you're trying to move through this material. As you move, you encounter these force fields created by the atoms. Instead of slowing you down, these force fields actually guide you along a specific path, almost like having a team of tiny helpers clearing the way for you!This effect was discovered by a group of brilliant scientists in China, and it's considered a huge breakthrough in the field of quantum physics (the study of really, really small things). It could lead to all sorts of amazing technologies, like super-fast computers and more efficient ways to transmit electricity.But that's not all! This discovery is also important because it proves that China is at the forefront of cutting-edge scientific research. The scientists who made this discovery are being hailed as potential Nobel Prize winners, which is one of the highest honors a scientist can receive.Isn't it amazing how these tiny, invisible particles can do such incredible things? The world of science is full ofmind-blowing discoveries, and the Quantum Anomalous Hall Effect is just one example of the amazing things that can happen when brilliant minds come together to explore the mysteries of the universe.So, the next time you hear someone mention the "Quantum Anomalous Hall Effect," you can proudly say, "Oh, I know all about that! It's a magical discovery that allows electricity to flow without any resistance, and it was made by amazing Chinese scientists!" Who knows, maybe one day you'll be the one making groundbreaking discoveries like this!。