A Twisted Factorization Method for Symmetric SVD of a Complex Symmetric Tridiagonal Matrix
Block-tridiagonalmatrices:块三对角矩阵

. – p.3/31
FMB - NLA Block-tridiagonal matrices
When the subdomains are ordered lexicographically from left to right, a domain Ω becomes coupled only to its pre- and postdecessors Ω 1 and Ω +1, respectively and the corresponding matrix takes the form of
. – p.4/31
FMB - NLA Block-tridiagonal matrices
How do we factorize a (block)-tridiagonal matrix ?
. – p.5/31
FMB - NLA
Let be block-tridiagonal, and expressed as =
...
76 76 76
12 ...
76
7
76
0
23 7
76
7
76 76 76
...
7 7 7
4
54
54
5
ÒÒ 1 0
1Ò
0
Factorization algorithm: 1 = 11
=
11
1
. – p.7/31
FMB - NLA = Ä 1Í for pointwise tridiagonal matrices
2
1
=
Á
4
0
32
11 12
11
54
Á
0
32 0
一种新的流变相法制备锂离子电池纳米_LiVOPO_4正极材料_英文_

一种新的流变相法制备锂离子电池纳米-LiVOPO 4正极材料熊利芝1,2何则强1,2,*(1吉首大学生物资源与环境科学学院,湖南吉首416000;2中南大学化学化工学院,长沙410083)摘要:采用新型流变相法制备锂离子电池正极材料纳米-LiVOPO 4.采用X 射线衍射、扫描电子显微镜以及电化学测试等手段对LiVOPO 4的微观结构、表面形貌和电化学性能进行了表征.结果表明,采用流变相法制备的LiVOPO 4由粒径大约在10-60nm 的小颗粒组成.首次放电容量,首次充电容量以及库仑效率分别为135.7mAh·g -1,145.8mAh ·g -1和93.0%.0.1C (1C =160mA ·g -1)放电时,60次循环后,放电容量保持在134.2mAh ·g -1,为首次放电容量的98.9%,平均每次循环的容量损失仅为0.018%.而1.0C 和2.0C 放电时的放电容量达到0.1C 放电容量的96.5%和91.6%.随着放电次数的增加,电荷转移阻抗增加,而锂离子在电极中的扩散系数达到10-11cm 2·s -1数量级.实验结果显示采用流变相法制备的LiVOPO 4是一种容量高、循环性能好、倍率性能好的锂离子电池正极材料.关键词:锂离子电池;流变相法;LiVOPO 4;倍率性能;扩散系数中图分类号:O646A New Rheological Phase Route to Synthesize Nano -LiVOPO 4Cathode Material for Lithium Ion BatteriesXIONG Li -Zhi 1,2HE Ze -Qiang 1,2,*(1College of Biology and Environmental Sciences,Jishou University,Jishou 416000,Hunan Province,P.R.China ;2College of Chemistry and Chemical Engineering,Central South University,Changsha 410083,P.R.China )Abstract :A novel lithium -ion battery cathode material,nano -LiVOPO 4,was synthesized by a new rheological phase method.The microstructure,surface morphology,and electrochemical properties were characterized by various electrochemical methods in combination with X -ray diffraction (XRD)and scanning electron microscopy (SEM).Results show that the orthorhombic LiVOPO 4,obtained by this rheological phase method,is made up of 10-60nm particles.The first discharge capacity,charge capacity,and columbic efficiency of LiVOPO 4were found to be 135.7mAh ·g -1,145.8mAh·g -1,and 93.0%,respectively.After 60cycles,the discharge capacity remained 134.2mAh ·g -1,at 98.9%of the first discharge capacity,and the capacity loss per cycle was only 0.018%at 0.1C (1C =160mA ·g -1).More than 96.5%and 91.6%of the discharge capacity at 0.1C were maintained at 1.0C and 2.0C ,respectively.The charge transfer resistance increased with the increase of the cycle number and the diffusion coefficient of lithium ion in the nano -LiVOPO 4was in the order of 10-11cm 2·s -1.Experimental results suggest that the rheological phase method is a good route for the synthesis of LiVOPO 4cathode material of high capacity,good cycling performance,and good current rate capability for lithium ion batteries.Key Words :Lithium ion battery;Rheological phase method;LiVOPO 4;Current rate capability;Diffusioncoefficient[Article]物理化学学报(Wuli Huaxue Xuebao )Acta Phys.-Chim.Sin .,2010,26(3):573-577March Received:October 18,2009;Revised:December 16,2009;Published on Web:January 13,2010.*Corresponding author.Email:csuhzq@;Tel:+86-743-8563911.The project was supported by the National Natural Science Foundation of China (20873054),Natural Science Foundation of Hunan Province,China (07JJ3014),Postdoctoral Science Foundation of China (2005037700),Scientific Research Fund of Hunan Provincial Education Department,China (07A058),and Postdoctoral Science Foundation of Central South University,China (2004107).国家自然科学基金(20873054)、湖南省自然科学基金(07JJ3014)、中国博士后科学基金(2005037700)、湖南省教育厅科研项目(07A058)和中南大学博士后科学基金(2004107)资助鬁Editorial office of Acta Physico -Chimica Sinica573Acta Phys.-Chim.Sin.,2010Vol.26Recently,performance of mobile electronic devices,such as mobile phone or laptop computer,is drastically improving and so,the demands for battery become more severe.Due to its large power density and cycle stability,lithium ion battery is now widely used for the electric source of mobile equipment.The current most important requirement for lithium ion rechargeable battery is to decrease cost and increase the power density.In the current battery,LiCoO2and graphitic carbon are commonly used for cathode and anode,respectively.However,natural abun-dance of Co is limited and this element is expensive.Therefore, development of cathode material without containing Co is strongly required.At present,great attentions are paid for tansi-tion metal phosphates,such as LiMPO4(M=Fe,Mn,Co)[1-4], Li3V2(PO4)3[5-10],and LiVPO4F[11-12],as a new class of cathode ma-terials for lithium ion batteries.These materials contain both mo-bile lithium ions and redox-active transition metals within a rigid phosphate network,and display remarkable electrochemical,and thermal stabilities as well as comparable energy density.Among these materials,LiFePO4is of great interest for the replacement of LiCoO2in Li ion batteries due to its low cost,nontoxicity and good electrochemical properties since1997[1,13-17].However,com-pared with LiFePO4,LiVOPO4has an advantage of higher poten-tial(4.0and3.7V(versus Li/Li+))for charge and discharge, and this phosphate is highly interesting from the viewpoint of the alternative cathode[18-21].Kerr et al.[22]presented that the tri-c linic phase LiVOPO4synthesized fromε-VOPO4showed the capacity of100mAh·g-1up to100cycles at C/10of current rate. Azmi et al.[19,23]reported that orthorhombic phase of LiVOPO4 could be synthesized by impregnation method and exhibited fairly good cycle stability for Li de-intercalation and intercala-tion.For all functional materials,their properties were greatly in-fluenced by the synthesis methods.Many preparation methods have been investigated with an aim to achieve high capacity LiVOPO4,however,the capacity of the products ever reported is usually unsatisfactory in particular when discharged at a high current rate.To meet high power demands of lithium ion batteries in new applications,the rate capability of LiVOPO4has to be sig-nificantly improved.There are two main frequently employed strategies:one is to increase the intrinsic electronic conductivity by microstructure controlling,the other is to enhance lithium ion transport by reducing the bulk diffusion length,which can be achieved by utilization of nanostructured materials.The rheological phase method is the process of preparing compounds or materials from a solid-liquid rheological mixture. That is,the solid reactants are fully mixed in a proper molar ratio, and made up by a proper amount of water or other solvents to form a Bingham body in which the solid particles and liquid substance are uniformly distributed,so that the product can be obtained under suitable experiment conditions[24].Because of its low temperature,short calcination time,and products with small particle with uniform distribution,rheological method has been used to synthesize cathode and anode materials for lithium ion batteries[25-26].In the present study,rheological technique is used to synthesize nano-LiVOPO4.The microstructure and electro-chemical properties of LiVOPO4as cathode material for lithium ion batteries were studied.1ExperimentalAnalytical grade powders of LiOH·2H2O(AR),NH4VO3(AR), (NH4)2HPO4(AR)and citric acid(AR)with equal amount of substance were mixed uniformly to get a mixture.Then1.5mL distilled water per gram mixture was added to the mixture under magnetic force stirring to obtain a mash.The mash was dried in vacuum at80℃for4h to form the precursor.The precursor was calcined in Ar atmosphere at650℃for6h to obtain blue LiVOPO4powders.Phase identification and surface morphology studies of the samples were carried out by an X-ray diffractometer(XRD;D/ MAX-gA,Rigaku Corporation,Japan)with Cu Kαradiation and scanning electron microscope(SEM;JSM5600LV,JEOL Ltd., Japan,accelerating voltage of20kV).Elemental analyses for lithium,vanadium,and phosphorus were determined by atomic absorption spectroscopy(AAS;SP-3530AA)and inductively cou-pled plasma-atomic emission spectrometer(ICP;TY9900).A slurry containing80%(mass fraction,similarly hereinafter) LiVOPO4,10%acetylene black,and10%PVDF(polyvinyli-dene fluoride)was made using N-methylprrolidinone(NMP)as the solvent.The electrodes with an area of1cm2were prepared by coating the slurry(about100μm in thickness)on alumin-um foils followed by drying in vacuum at60℃for12h.Elec-trochemical tests were performed using a conventional cointype cell,employing lithium foil as a counter electrode and1.0mol·L-1LiPF6in ethylene carbonate/dimethyl carbonate(EC/DMC) (with EC and DMC volume ratio of1∶1)as the electrolyte.The assembly was carried out in an Ar-filled glove box.The electr-ochemical tests were carried out with an electrochemical work station(CHI660B,CHI Instruments Inc.,Shanghai,China).2Results and discussionFig.1shows the XRD pattern of LiVOPO4derived from rheo-logical phase method.As shown in Fig.1(a),All the reflections from the LiVOPO4could be indexed reliably using a standard structural refinement program.XRD peaks in Fig.1agree well with those of the standard JCPDS card No.72-2253.The LiVOPO4 compound possesses an orthorhombic symmetry,space group Pnma,characterized by the unit cell parameters a=0.7446(4) nm,b=0.6278(4)nm,and c=0.7165(4)nm.Except for peaks corresponding to LiVOPO4,no other peaks can be found,sug-gesting that the rheologically synthsized LiVOPO4is very pure. The LiVOPO4framework structure is closely related to that found in VOPO4and comprises infinite chains of corner-shared VO6 octahedra,cross-linked by corner-sharing PO4tetrahedron[27-28]. The cell parameters for the rheologically prepared material com-pare favorably with literature values reported by Lii et al.[28]for a574No.3XIONG Li-Zhi et al.:A New Rheological Phase Route to Synthesize Nano-LiVOPO4Cathode MaterialFig.1XRD pattern(a)and SEM image(b)of LiVOPO4hydrothermally prepared sample,i.e.,a=0.7446(3)nm,b=0.6292(2) nm,and c=0.7177(2)nm.Elemental analysis results confirmed the expected stoichiometry of LiVOPO4.As seen from Fig.1(b),the scanning electron microscopy(SEM) examination indicated that the rheologically synthsized LiVOPO4 consists of particles with average primary size in the range of 10-60nm,which agrees well with the average crystal size of around35nm calculated from the XRD profile.They also showed the presence of considerable material agglomeration. The agglomerates averaged around50nm in size.The lithium extraction/insertion behavior for the LiVOPO4 active material relies on the reversibility of the V4+/V5+redox couple:LiVOPO4圳VOPO4+Li++e-Fig.2shows the initial charge-discharge curve of the rheologi-cally synthesized LiVOPO4material.These data were collected at25℃at an approximate0.1C(16mA·g-1)rate using voltage limits of3.0and4.3V(vs Li/Li+).As shown in Fig.2,at low current density,orthorhombic LiVOPO4prepared by rheological phase method is highly attractive as the alternative cathode for lithium ion rechargeable battery.This is because LiVOPO4ex-hibits high discharge potential of3.85V and reasonably large capacity.The initial oxidation process equates to a material spe-cific capacity of145.8mAh·g-1during this lithium extraction. Based on a theoretical specific capacity for LiVOPO4of166 mAh·g-1[20]and assuming no side reactions,the fully charged material corresponds to Li0.12VOPO4.Excursions to higher oxi-dation potentials(ultimately up to5.0V(vs Li/Li+))resulted in the increased irreversibility as well as active material degrada-tion evidenced by electrolyte discoloration.The reinsertion pro-cess amounts to135.7mAh·g-1,indicating a higher first-cycle charge reversibility of93%than the literature value(85%)repor-ted by Barker et al.[29].The cycling performance was tested at0.1C(16mA·g-1)in the range of3.0-4.3V as shown in the insert figure in Fig.2.Af-ter cycling60times,the discharge capacity of LiVOPO4is sus-tained at134.2mAh·g-1,which is98.9%of the initial capacity,and the capacity loss per cycle is only0.018%,suggesting LiVOPO4 derived by rheological phase method is promising as alternative cathode material for lithium ion batteries with high capacity and good cycling performance.Fig.3shows the discharge capacity of LiVOPO4as a function of current rate.As shown in Fig.3,discharge capacity of LiVOPO4drastically decreased with increasing current rate due to the increase of the polarization of electrode.The discharge capacity of LiVOPO4at0.1C(16mA·g-1),1.0C(160mA·g-1),Fig.2Electrochemical performance data for a typical Li/ LiVOPO4cell cycled between3.0and4.3V at approximate0.1C(16mA·g-1)rate for charge and dischargeThe inset figure in Fig.2is the cycling performance curve.Fig.3Discharge capacity of LiVOPO4as a function ofcurrent ratepotential window:3.0-4.3V(vs Li/Li+);1C=160mA·g -1575Acta Phys.-Chim.Sin.,2010Vol.26Fig.4i-t(a)and i-t-1/2(b)curves of nano-LiVOPO4electrodeFig.5Electrochemical impedance spectroscopy of nano-LiVOPO4electrode at various cycling timesIn the equivalent circuit,R e is the electrolyte resistance,R ct is the charge-transfer resistance,C dl is the double layer capacitance,Z w is the Warburg impedance,andC L is the intercalation capacitance.and2.0C(320mA·g-1)is135.7,130.9,and124.3mAh·g-1,resp-ectively.More than96.5%and91.6%of the discharge capacity at0.1C are sustained at1.0C and2.0C,respectively.This result is better than that of the LiVOPO4reported by Azmi et al.[19],in-dicating good current rate capability of LiVOPO4synthesized by rheological phase method.The good current rate capability may result mainly from the small particle size and large surface area of LiVOPO4nanoparticles.The smaller the particle size,the larger the surface area and the lower the current density,which results in less polarization of electrode and better current rate capability of LiVOPO4.Further work is underway to find out if there are any other reasons leading to good current rate capabili-ty of LiVOPO4.The chemical diffusion coefficient was measured with the po-tential step technique.In this method,the current generated due to an applied voltage step,is measured as a function of time. The measured current decays as the lithium ion diffuses throughthe electrode.The step ends when the current becomes less than 1%of the maximum current at the onset of the applied potential. The i-t and i-t-1/2curves for the two powders at the applied po-tential step of0.1V(vs Li/Li+)(3.94→4.04V)are shown in Fig.4.By assuming that the semi-finite diffusion of lithium ion in the electrode is the rate-determining procedure,the diffusion coefficient(D)of lithium ion in the electrode can be determined by the following Cottrell equation[30]:i=nFD1/2c0π-1/2t-1/2where,n is the number of the redox reactions,F is the Faraday constant,and c0is the lithium ion concentration in the solid electrode,which can be calculated from the open circuit voltage. According to Fig.4and Cottrell equation,the diffusion coeffi-cient of lithium ion in the electrode can be calculated to be 5.52×10-11cm2·s-1,which is as same magnitude again as the value(2.79×10-11cm2·s-1)reported by Ren et al.[20].The experi-ment results show that the current rate capability of LiVOPO4by rheological phase method is better than that reported by Azmi et al.[19],while the diffusion coefficient of lithium ion in the elec-trode is in the same order.This may be due to the difference in preparation methods of materials and testing means of diffusion coefficient.The electrochemical impedance spectroscopy of nano-LiVOPO4and the equivalent circuit are displayed in Fig.5.All the spectra show a semicircle in the high frequency range and an inclined line in the low frequency range.The semicircle in the high frequency range is associated with the“charge trans-fer reactions”at the interface of electrolyte/oxide electrode,which corresponds to the charge transfer resistance.The inclined line in the low frequency range is attributable to“Warburg impedan-ce”that is associated with lithium ion diffusion through the oxide electrode.The semicircle increases with the increase of cycle number.This indicates that the“charge transfer”resistance be-comes larger with the increase of cycle number.The figure also shows that the slope of the inclined line varies with the cycle number.The slope of the inclined line at the first cycle is the biggest and after cycling10times it gets smaller.However, when the cycle number reaches60,the slope of the inclined line becomes stable.3Conclusions(1)Orthorhombic nano-LiVOPO4with particle size in the range of10-60nm was synthesized by a new rheological phase method.(2)The first discharge of LiVOPO4is135.7mAh·g-1and 98.9%of that is kept after60cycles.More than96.5%and576No.3XIONG Li-Zhi et al.:A New Rheological Phase Route to Synthesize Nano-LiVOPO4Cathode Material91.6%of the discharge capacity at0.1C are sustained at1.0C and2.0C,respectively.The chemical diffusion coefficient of lithium ion in the nano-LiVOPO4was measured with the potential step technique and the value is in the order of10-11 cm2·s-1.(3)Rheological phase method is a good route to synthesize LiVOPO4cathode material with high capacity,good cycling performance,and good current rate capability for lithium ion batteries.References1Padhi,A.K.;Najundaswamy,K.S.;Goodenough,J.B.J.Electrochem.Soc.,1997,144:11882Yamada,A.;Chung,S.C.J.Electrochem.Soc.,2001,148:A960 3Amine,K.;Yasuda,H.;Yamachi,M.Electrochem.Solid State Lett.,2000,3:1784Azuma,G.;Li,H.;Tohdam,M.Electrochem.Solid State Lett., 2002,5:A1355Saidi,M.Y.;Barker,J.;Huang,H.;Sowyer,J.L.;Adamson,G.J.J.Power Sources,2003,119-112:2666Yin,S.C.;Grond,H.;Strobel,P.;Huang,H.;Nazar,L.F.J.Am.Chem.Soc.,2003,125:3267Hung,H.;Yin,S.C.;Kerr,T.;Taylor,N.;Nazar,L.F.Adv.Mater., 2002,14:15258Ren,M.M.;Zhou,Z.;Li,Y.Z.;Gao,X.P.;Yan,J.J.Power Sources,2006,162:13579Li,Y.Z.;Zhou,Z.;Ren,M.M.;Gao,X.P.;Yan,J.Electrochim.Acta,2006,51:649810Ren,M.M.;Zhou,Z.;Gao,X.P.;Peng,W.X.J.Phys.Chem.C, 2008,112:568911Barker,J.;Saidi,M.Y.;Swoyer,J.L.J.Electrochem.Soc.,2003, 150:A139412Li,Y.Z.;Zhou,Z.;Gao,X.P.;Yan,J.J.Power Sources,2006, 160:63313Yamada,A.;Chung,S.C.;Hinokuma,K.J.Electrochem.Soc.,2001,148:A22414Andersson,A.S.;Thomas,J.O.;Kalska,B.;Haggstrom,L.Electrochem.Solid State Lett.,2000,3:6615Konarova,M.;Taniguchi,I.J.Power Sources,2009,194:1029 16Kuwahara,A.;Suzuki,S.;Miyayama,M.Ceramics International, 2008,34:86317Li,J.;Suzuki,T.;Naga,K.;Ohzawa,Y.;Nakajima,T.Mater.Sci.Eng.B-Solid State Mater.Adv.Technol.,2007,142:8618Azmi,B.M.;Ishihara,T.;Nishiguchi,H.;Takita,Y.Electrochim.Acta,2002,48:16519Azmi,B.M.;Ishihara,T.;Nishiguchi,H.;Takita,Y.J.Power Sources,2005,146:52520Ren,M.M.;Zhou,Z.;Su,L.W.;Gao,X.P.J.Power Sources, 2009,189:78621Yang,Y.;Fang,H.;Zheng,J.;Li,L.;Li,G.;Yan,G.Solid State Sciences,2008,10:129222Kerr,T.A.;Gaubicher,J.;Nazar,L.F.Electrochem.Solid State Lett.,2000,3:46023Azmi,B.M.;Ishihara,T.;Nishiguchi,H.;Takita,Y.Electrochemistry,2003,71:110824Sun,J.;Xie,W.;Yuan,L.;Zhang,K.;Wang,Q.Mater.Sci.Eng.B-Solid State Mater.Adv.Technol.,1999,64:15725He,Z.Q.;Li,X.H.;Xiong,L.Z.;Wu,X.M.;Xiao,Z.B.;Ma,M.Y.Materials Chemistry and Physics,2005,93:51626He,B.L.;Zhou,W.J.;Bao,S.J.;Liang,Y.Y.;Li,H.L.Electrochim.Acta,2007,52:328627Gaubicher,J.;Orsini,F.;Le Mercier,T.;Llorente,S.;Villesuzanne,A.;Angenault,J.;Quarton,M.J.Solid State Chem.,2000,150:25028Lii,K.H.;Li,C.H.;Cheng,C.Y.;Wang,S.L.J.Solid State Chem.,1991,95:35229Barker,J.;Saidi,M.Y.;Swoyer,J.L.J.Electrochem.Soc.,2004, 151:A79630Bard,A.J.;Faulkner,L.R.Electrochemical methods:fundamentals and applications.2nd ed.New York:Wiley,2001577。
A Weierstrass-type theorem for homogeneous polynomials

a rX iv:mat h /51367v1[mat h.CA]18O ct25A Weierstrass-type theorem for homogeneous polynomials David Benko,Andr´a s Kro´o †February 2,2008Abstract By the celebrated Weierstrass Theorem the set of algebraic polyno-mials is dense in the space of continuous functions on a compact set in R d .In this paper we study the following question:does the density hold if we approximate only by homogeneous polynomials?Since the set of homogeneous polynomials is nonlinear this leads to a nontrivial problem.It is easy to see that:1)density may hold only on star-like 0-symmetric surfaces;2)at least 2homogeneous polynomials are needed for approxi-mation.The most interesting special case of a star-like surface is a convex surface.It has been conjectured by the second author that functions con-tinuous on 0-symmetric convex surfaces in R d can be approximated by a pair of homogeneous polynomials.This conjecture is not resolved yet but we make substantial progress towards its positive settlement.In particu-lar,it is shown in the present paper that the above conjecture holds for 1)d =2,2)convex surfaces in R d with C 1+ǫboundary.1Introduction The celebrated theorem of Weierstrass on the density of real algebraic polyno-mials in the space of real continuous functions on an interval [a,b ]is one of the main results in analysis.Its generalization for real multivariate polynomi-als was given by Picard,subsequently the Stone-Weierstrass theorem led to the extension of these results for subalgebras in C (K ).In this paper we shall consider the question of density of homogeneous poly-nomials .Homogeneous polynomials are a standard tool appearing in many areas of analysis,so the question of their density in the space of continuous functions is a natural problem.Clearly,the set of homogeneous polynomials is substantially smaller relative to all algebraic polynomials.More importantly,this set is nonlinear,so its density can not be handled via the Stone-Weierstrass theorem.Furthermore,due to the special structure of homogeneous polynomi-als some restrictions should be made on the sets were we want to approximate (they have to be star-like),and at least2polynomials are always needed for approximation(an even and an odd one).On the5-th International Conference on Functional Analysis and Approxi-mation Theory(Maratea,Italy,2004)the second author proposed the following conjecture.Conjecture1Let K⊂R d be a convex body which is centrally symmetric to the origin.Then for any function f continuous on the boundary Bd(K)of K and anyǫ>0there exist two homogeneous polynomials h and g such that |f−h−g|≤ǫon Bd(K).From now on we agree on the terminology that by“centrally symmetric”we mean“centrally symmetric to the origin”.Subsequently in[4]the authors verified the above Conjecture for crosspoly-topes in R d and arbitrary convex polygons in R2.In this paper we shall verify the Conjecture for those convex bodies in R d whose boundary Bd(K)is C1+ǫfor some0<ǫ≤1(Theorem2).Moreover, the Conjecture will be verified in its full generality for d=2(Theorem3).It should be noted that parallel to our investigations P.Varj´u[13]also proved the Conjecture for d=2.In addition,he gives in[13]an affirmative answer to the Conjecture for arbitrary centrally symmetric polytopes in R d,and for those convex bodies in R d whose boundary is C2and has positive curvature.We also would like to point out that our method of verifying the Conjecture for d=2is based on the potential theory and is different from the approach taken in[13] (which is also based on the potential theory).Likewise our method of treating C1+ǫconvex bodies is different from the approach used in[13]for C2convex bodies with positive curvature.2Main ResultsLet K be a centrally symmetric convex body in R d.We may assume that 2≤d and dim(K)=d.The boundary of K is Bd(K)which is given by the representationBd(K):={u r(u):u∈S d−1}where r is a positive even real-valued function on S d−1.Here S d−1stands for the unit sphere in R d.We shall say that K is C1+ǫ,written K∈C1+ǫ,if the first partial derivatives of r satisfy a Lipǫproperty on the unit sphere,ǫ>0. Furthermore denote byc k x k:c k∈RH d n:=k1+...+k d=nthe space of real homogeneous polynomials of degree n in R d.Ourfirst main result is the following.2Theorem2Let K∈C1+ǫbe a centrally symmetric convex body in R d,where 0<ǫ≤1.Then for every f∈C(Bd(K))there exist h n∈H d n+H d n−1,n∈N such that h n→f uniformly on Bd(K)as n→∞.Thus Theorem2gives an affirmative answer to the Conjecture under the additional condition of C1+ǫsmoothness of the convex surface.For d=2we can verify the Conjecture in its full generality.Thus we shall prove the following. Theorem3Let K be a centrally symmetric convex body in R2.Then for every f∈C(Bd(K))there exist h n∈H2n+H2n−1,n∈N such that h n→f uniformly on Bd(K)as n→∞.We shall see that Theorem3follows fromTheorem4Let1/W(x)be a positive convex function on R such that|x|/W(−1/x)is also positive and convex.Let g(x)be a continuous function which has the same limits at−∞and at+∞.Then we can approximate g(x) uniformly on R by weighted polynomilas W(x)n p n(x),n=0,2,4,...,deg p n≤n.3Proof of Theorem2The proof of Theorem2will be based on several lemmas.The main auxiliary result is the next lemma which provides an estimate for the approximation of unity by even homogeneous polynomials.In what follows||...||D stands for the uniform norm on D.Our main lemma to prove Theorem2is the following.Lemma5Letτ∈(0,1).Under conditions of Theorem2there exist h2n∈H d2n,n∈N,such that||1−h2n||Bd(K)=o(n−τǫ).The next lemma provides a partition of unity which we shall need below. In what follows a cube in R d is called regular if all its edges are parallel to the coordinate axises.We denote the set{0,1,2,...}d by Z d+.Lemma6Given0<h≤1there exist non-negative even functions g k∈C∞(R d)such that their support consists of2d regular cubes with edge h,at most2d of supports of g k’s have nonempty intersection,andg k(x)=1,x∈R d,(1)k∈Z d+|∂m g k(x)/∂x m j|≤c/h m,x∈R d,m∈Z1+,1≤j≤d,(2) where c>0depends only on m∈Z1+and d.3For the centrally symmetric convex body K let|x|K:=inf{a>0:x/a∈K}be its Minkowski functional and setδK:=sup{|x|/|x|K:x∈R d}=max{|x|:x∈Bd(K)}. Moreover for a∈Bd(K)denote by L a a supporting hyperplane at a.Lemma7Let a∈Bd(K),h n∈H d2n be such that for any x∈L a,|x−a|≤4δK we have|h n(x)|≤1.Then whenever x∈L a satisfies|x−a|>4δK and x/t∈K we have|h n(x/t)|≤(2/3)2n.(3) Lemma8Consider the functions g k from Lemma6.Then for at most8d/2h d of them their support has nonempty intersection with S d−1.We shall verifyfirst the technical Lemmas6-8,then the proof of Lemma 5will be given.Finally it will be shown that Theorem2follows easily from Lemma5.Proof of Lemma6.The main step of the proof consists of verifying the lemma for d=1.Let g∈C∞(R)be an odd function on R such that g=1for x<−1/2and monotone decreasing from1to0on(−1/2,0).Further,let g∗(x) be an even function on R such that g∗(x)equals1on[0,1],g(x−3/2)/4+3/4on [1,2],and g(x−5/2)/4+1/4on[2,3].Then it is easy to see that g∗∈C∞(R),it equals1on[−1,1],0for|x|>3and is monotone decreasing on[1,3].Moreoverg∗(x)+g∗(x−4)=1,x∈[−1,5].(4) Set nowg k(x):=g∗(x−4k)+g∗(x+4k),k∈Z1+.Then g k’s are even functions which by(4)satisfy relation∞k=0g k(x)=1,x∈R.In addition,the support of g k equals±[−3+4k,3+4k]and at most2of g k’s can be nonzero at any given x∈R.Finally,for afixed0<h≤1,x∈R d and k=(k1,...,k d)∈Z d+setg k(x):=dj=1g kj(6x j/h).It is easy to see that these functions give the needed partition of unity.Proof of Lemma7.Clearly the conditions of lemma yield that whenever |x−a|>4δK1/|x|K≤δK/|x|≤δK/(|x−a|−|a|)≤δK/(|x−a|−δK)≤4δK/3|x−a|.(5) It is well known that for any univariate polynomial p of degree at most n such that|p|≤1in[−a,a]it holds that|p(x)|≤(2x/a)n whenever|x|>a.Therefore using(5)and the assumption imposed on h n we have|h n(x)|≤(2|x−a|/4δK)2n≤(2|x|K/3)2n.(6) Now it remains to note that by x/t∈K it follows that|x|K≤|t|,and thus we obtain(3)from(6).This completes the proof of the lemma.Proof of Lemma5.Denote by g k,1≤k≤N those functions from Lemma 6whose support A k has a nonempty intersection with S d−1.Then by Lemma8N≤8d/2h d.(9) Moreover,by(1)Ng k=1on S d−1.(10)k=1SetB k:=A k∩S d−1,C k:={u r(u):u∈B k}⊂Bd(K),1≤k≤N. For each1≤k≤N choose a point u k∈B k and set x k:=u k r(u k)∈Bd(K). Furthermore let L k be the supporting plane to Bd(K)at the point x k and set for1≤k≤N,L∗k:=L k∪(−L k)D k:={x∈L∗k:x=t u for some u∈B k,t>0};5f k(x):=g k(u),x∈Bd(K),x=u r(u),u∈S d−1q k(x):=g k(u),x∈L∗k,x=t u,u∈S d−1,t>0. Clearly,q k∈C∞(L∗k)is an even positive function which by property(2)canbe extended to a regular centrally symmetric cube I⊃K so that we have on I |∂m q k/∂x m j|≤c/h m,1≤j≤d,1≤k≤N.(11) Here and in what follows we denote by c(possibly distinct)positive constants depending only on d,m and K.We can assume that I is sufficiently large so thatI⊃G k:={x∈L k:|x−x k|≤4δK},1≤k≤N.Then by the multivariate Jackson Theorem(see e.g.[10])applied to the even functions q k satisfying(11)for arbitrary m∈N(to be specified below),there exist even multivariate polynomials p k of total degree at most2n such that||q k−p k||G∗k≤c/(hn)m≤1,1≤k≤N,(12) where G∗k:=G k∪(−G k),h:=n−γ(0<γ<1is specified below),and n is sufficiently large.We claim now that without loss of generality it may be assumed that each p k is in H d2n.Indeed,since G∗k⊂L∗k it follows that the homogeneous polynomial h2:=<x,w>2∈H d2is identically equal to1on G∗k(here w is a properly normalized normal vector to L k),so multiplying the even degree monomials of p k by even powers of h2we can replace p k by a homogeneous polynomial from H d2n so that(12)holds.Thus we may assume that p k∈H d2n and relations(12) hold.In particular,(12)also yields that||p k||G∗k≤2,1≤k≤N.(13) Now consider an arbitrary x∈Bd(K)\C k.Then with some t>1we have t x∈L∗k and q k(t x)=0.Hence if t x∈G∗k then by(12)it follows that|p k(x)|≤|p k(t x)|≤c/(hn)m.On the other hand if t x/∈G∗k then by(13)and Lemma7we obtain|p k(x)|≤2(2/3)2n.The last two estimates yield that for every x∈Bd(K)\C k we have|p k(x)|≤c((2/3)2n+(hn)−m),1≤k≤N.(14) Now let us assume that x∈C k.Clearly,the C1+ǫproperty of Bd(K)yields that whenever x∈Bd(K),t x∈L∗k,t>1we have for every1≤k≤N(t−1)|x|=|x−t x|≤c min{|x−x k|,|x+x k|}1+ǫ.(15)6Obviously,for every u∈B kmin{|u−u k|,|u+u k|}≤√1+m+ǫ+d >τǫand letγ:=1+mProof of Theorem2.First we use the classical Weierstrass Theorem to approximate f∈C(Bd(K))by a polynomialp m=mj=0h∗j,h∗j∈H d j,0≤j≤mof degree at most m so that||f−p m||Bd(K)≤δ7with any givenδ>0.Letτ∈(0,1)be arbitrary.According to Lemma5 there exist h n,j∈H d2n−2[j/2]such that||1−h n,j||Bd(K)=O(n−τǫ),0≤j≤m.Clearly,h∗:=mj=0h n,j h∗j∈H d2n+H d2n+1and||f−h∗||Bd(K)≤δ+O(n−τǫ).W(t)is positive and convex on R(19)|t|t )is positive and convex on R.(20)Remark10Equivalently,instead of(20)we may assume that(21)below holds and lim t→+∞t(tQ′(t)−1)≤lim t→−∞t(tQ′(t)−1).We also remark that(19) implies that(20)is satisfied on(−∞,0)and on(0,+∞).We mention the function W(t)=(1+|t|m)−1/m,1≤m,as an example which satisfies(19)and(20).We say that a property is satisfied inside R if it is satisfied on all compact subsets of R.Some consequences of(19)and(20)are as follows.8limt→±∞|t|W(t)=ρ∈(0,+∞)exists.(21) Since exp(Q(t))is convex,it is Lipschitz continuous inside R.So exp(Q(t)) is absolutely continuous inside R which implies that both W(t)and Q(t)are absolutely continuous inside R.Q′(t)is bounded inside R a.e.because by(19)exp(Q(t))Q′(t)is increasing a.e.We collected below some frequently used definitions and notations in the paper.Definitions11Let L⊂R and let f:L→R∪{−∞}∪{+∞}.f is H¨o lder continuous with H¨o lder index0<τ≤1if with some K constant |f(x)−f(y)|≤K|x−y|τ,x,y∈L.In this case we write f∈Hτ(L).The L p norm of f is denoted by||f||p.When p=∞we will also use the ||f||L notation.We say that an integral or limit exists if it exists as a real number.Let x∈R.If f is integrable on L\(x−ǫ,x+ǫ)for all0<ǫthen the Cauchy principal value integral is defined asP V L f(t)dt:=limǫ→0+ L\(x−ǫ,x+ǫ)f(t)dt,if the limit exists.It is known that P V L g(t)/(t−x)dt exists for almost every x∈R if g: L→R is integrable.For0<ιand a∈R we definea+ι:=max(a,ι)and a−ι:=max(−a,ι).For a>b the interval[a,b]is an empty set.We say that a property is satisfied inside L if it is satisfied on all compact subsets of L.o(1)will denote a number which is approaching to zero.For example,we may write10x=100+o(1)as x→2.Sometimes we also specify the domain(which may change withǫ)where the equation should be considered.For example, sin(x)=o(1)for x∈[π,π+ǫ]whenǫ→0+.The equilibrium measure and its support S w is defined on the next page.Let [aλ,bλ]denote the support S Wλ(see Lemma(15)).For x∈(aλ,bλ)let Vλ(x):=0,and for a.e.x∈(aλ,bλ)letVλ(x):=P V bλaλλ√t−x dt(x−aλ)(bλ−x)+1(x−aλ)(bλ−x).(22)Let x∈[−1,1].Depending on the value of c∈[−1,1]the following integrals may or may not be principal value integrals.v c(x):=−P V c−1λ√π2√h c(x):=P V 1cλ√π2√1−t2e−Q(t)dt,x∈[−1,1].1−x2(t−x)P n(x)and p n(x)denote polynomials of degree at most n.Functions with smooth integrals was introduced by Totik in[11].Definitions12We say that f has smooth integral on R⊂L,if f is non-negative a.e.on R andf=(1+o(1)) J f(23)Iwhere I,J⊂R are any two adjacent intervals,both of which has length0< epsilon,andǫ→0.The o(1)term depends onǫand not on I and J.We say that a family of functions F has uniformly smooth integral on R, if any f∈F is non-negative a.e.on R and(23)holds,where the o(1)term depends onǫonly,and not on the choice of f,I or J.Cleary,if f is continuous and it has a positive lower bound on R then f has smooth integral on R.Also,non-negative linear combinations offinitely many functions with smooth integrals on R has also smooth integral on R.From the Fubini Theorem it follows that ifνis afinite positive Borel measure on T⊂R and{v t(x):t∈T}is a family of functions with uniformly smooth integral on R for which t→v t(x)is measureable for a.e.x∈[a,b],thenv(x):= T v t(x)dν(t)has also smooth integral on R.Finally,if f n→f uniformly a.e.on R,f n has smooth integral on R and f has positive lower bound a.e.on R then f has smooth integral on R. Remark13Since exp(−Q)is absolutely continuous inside R and(exp(−Q))′=−exp(−Q)Q′is bounded a.e.on[−1,1],by the fundamental theorem of calcu-√1+t∈H0.5([−1,1]), lus we see that exp(−Q(t))∈H1([−1,1]).And√1+t exp(−Q(t))∈H0.5([−1,1])so√soLet w(x)≡0be a non-negative continuous function on¯R such thatlimx→∞|x|w(x)=α∈[0,+∞)exists.(24) Whenα=0,then w belongs to the class of so called“admissible”weights.We write w(x)=exp(−q(x))and call q(x)externalfield.Ifµis a positive Borel unit measure on¯R-in short a“probability measure”,then its weighted energy is defined byI w(µ):= log1|t−x|dµ(t).This definition makes sense for a signed measureν,too,if log|t−x| d|ν|(t) exists.LetS w:=supp(µw)denote the support ofµw.Whenα=0,then S w is a compact subset of R.In this case with some F w constant we haveUµw+Q(x)=F w,x∈S w.Let Bd(K)be the boundary of a two dimensional convex region K⊂R2 which is centrally symmetric to the origin(0,0).For t∈R let(x(t),y(t))be any of the two points on Bd(K)for whichy(t)Lemma14W(t)satisfies properties(19),(20).And S W=¯R.Proof.W is positive on R.We may assume that x(t)>0,t∈R.Let t1,t3∈R and t2:=αt1+(1−α)t3,where0<α<1.Let(x2,y2)be the intersection of the line segments(0,0)(x(t2),y(t2)).Note that1/x(t2)≤1/x2and by elementary calculations:1x(t1)+(1−α)1Lemma15Let1<λ.Then S Wλis afinite interval[aλ,bλ],andµWλis absolutely continuous with respect to the Lebesgue measure and its density is dµWλ(x)=Vλ(x)dx.Proof.Let1<p.Note that exp(λQ(x))is a convex function because it is the composition of two continuous convex functions.So by[2],Theorem5, S Wλis an interval[aλ,bλ],which isfinite since lim x→±∞|x|Wλ(x)=0.The density function(dµWλ(x))/dx exists,since(Wλ)′=−exp(−λQ)λQ′∈L p(R),see Theorem IV.2.2of[8].The integral at(22)is the Hilbert transform on R of the function defined as λF Wλ,x∈[aλ,bλ],we get Uµ(x)=UµWλ(x),x∈(aλ,bλ).But(27)shows that Uµ+(x)and Uµ−(x)arefinite for all x∈[aλ,bλ].So Uµ+(x)=UµWλ+µ−(x), x∈(aλ,bλ).Hereµ+andµWλ+µ−are positive measures which have the same mass.µWλ,µ−(andµ+)all havefinite logarithmic energy(see(27)),henceµWλ+µ−has it,too.Applying Theorem II.3.2.of[8]we get Uµ+(z)=UµWλ+µ−(z)for all z∈C.By the unicity theorem([8],Theorem II.2.1.)µ+=µWλ+µ−.Henceµ=µWλand our lemma is proved.||µW [−N,N]||.We remark thatµW({∞})=0which implies that||µW [−N,N]||→1as N→+∞.(29) By([9],pp.3)there exists K∈R such that1K≤log|z−t|Wλ1(z)Wλ1(t)dνN(t)dνN(z)isfinite.(31) By(30)the double integral at(31)is bounded from below.It equals to: log1Here the first double integral is finite because V W is finite ([9],Theorem1.2).And thesecondintegral is bounded from above since νN has compact support.So (31)is established.Choose 0<τsuch that ||τW (x )||∞≤1.Now,I W (µ)−log(τ2)=lim M →+∞ min M,log 1|z −t |(τW (z ))(τW (t ))dµW λn (t )dµW λn (z )≤lim n →+∞ log 1|z −t |(τW (z ))λn (τW (t ))λndνN (t )dνN (z )= log 1|z −t |W (z )W (t )−K dνN (t )dνN (z )≤ log 1||µW[−N,N ]||dµW (z )||µW [−N,N ]||2 +1λ2ωS W λ S W λ2,14whereωSWλis the classical equilibrium measure of the set S Wλ(with no externalfield present).(We remark that S Wλ⊃SWλ2.)It follows that ifλis so close to1that SWλ2⊃I holds,then[a,b]⊂(aλ,bλ)and Vλ(x)has positive lower bound a.e.on[a,b].c−x,as x→c−.Here o(1)depends on c−x only.Lemma18Let−1<a<b<1and0<ιbefixed.Let0<ǫ<1/10and δ:=√v c(x2)+ι,v c(x1)−ιh c(x2)+ι,h c(x1)−ιt−x2≤1+x2−x1t−x1=(1+o(1))11−t2exp(−Q(t))/π2and integrating on [c,1]we gainh c(x2)1−x22/v c(x1)+ι=1+o(1).(34)Returning to the case of x1,x2≤c−δ,from v c(x)=h c(x)+B(x),from (33)and from B(x2)=B(x1)+o(1)we get|v c(x2)−v c(x1)|=|o(1)|(1+|v c(x1)−B(x1)|)≤|o(1)|(|v c(x1)|+1+||B||[a,b]).(35)15Assuming|v c(x1)|≤1,we have|v c(x2)+ι−v c(x1)+ι|≤|v c(x2)−v c(x1)|≤|o(1)|,so(34)holds again.Finally,if|v c(x1)|≥1,then from(35)v c(x2)|v c(x1)|=|o(1)|,from which(34)again easily follows.The proof of the rest of our lemma is similar.J v c(t)+ιdt=1+o(1),asǫ→0+,where o(1)is independent of I,J and c.Letδ:=√ǫ,c+√1−t2exp(−Q(t))/π2.Applying Lemma17(with A:=(a−1)/2,B:=(b+1)/2)we have√ǫ,c)asǫ→0+,which easily leads toh c(x)=(f(c)1−c2+o(1))(−log|c−x|)for x∈[c−√√ǫ,c)asǫ→0+.(36)Clearly,(36)also holds for x∈(c,c+√J v c(t)+ιdt=(f(c)1−c2+o(1)) I log1(f(c)1−c2+o(1)) J log1where we used that log(1/|x|)has smooth integral on[−2,2]([1],Proposition20).Following the proof of Lemma24of[1]we will prove the following lemma. But we remark that the absolutely continuous hypothesis of Lemma24is un-necessary at[1].Lemma21Let N(x)be a bounded,increasing,right-continuous function on [−1,1]and let f(x)∈L1([−1,1])be non-negative.ThenP V 1−1f(t)N(t)t−xdt, a.e.x∈[−1,1].Proof.Let us denote the left hand side of(38)by F(x).Since f(x)and f(x)N(x)are in L1[−1,1]and N(x)is increasing,there is a set of full mea-sure in(−1,1)where f1(x),F(x)and N′(x)all exist.Let x be chosen from this set.It follows that f c(x)exist for all c∈[−1,1]\{x}.Also,F(x)=limǫ→0+ x−ǫ−1f(t)N(t)t−xdt .(39) 17t→f t(x)is a continuous increasing function on[−1,x)and it is a continuous decreasing function on(x,1]so at(39)we can use integration by parts to get x−ǫ−1+ 1x+ǫ=−f x−ǫ(x)N(x−ǫ)+f−1(x)N(−1)+ (−1,x−ǫ]f t(x)dN(t)+f x+ǫ(x)N(x+ǫ)−f1(x)N(1)+ (x+ǫ,1]f t(x)dN(t)But above f−1(x)=0andf x+ǫ(x)N(x+ǫ)−f x−ǫ(x)N(x−ǫ)=[f x+ǫ(x)−f x−ǫ(x)]N(x+ǫ)+f x−ǫ(x)[N(x+ǫ)−N(x−ǫ)].(40) Note thatf x+ǫ(x)−f x−ǫ(x)=−P V x+ǫx−ǫf(t)Lemma22Let[a,b]be arbitrary and let1<λbe chosen to satisfy the conclu-sion of Lemma16.Then Vλ(x)has smooth integral on[a,b].Proof.To keep the notations simple we will assume that−1<a<b<1, and aλ=−1,bλ=1,that is,the support ofµWλis[−1,1].This can be done without loss of generality.Definev(t):=λ√π2√where v(t)also depends on the choice of x.Note that M(t),t∈[−1,1],is a bounded,increasing,right-continuous function which agrees with exp(Q(t))Q′(t) almost everywhere.Applying Lemma21for f(t):=v(t)and N(t):=M(t),let usfix an x∈[a,b] value for which both(38)and dµWλ(x)=Vλ(x)dx are satisfied.(These are satisfied almost everywhere.)From(22)and Lemma21we haveVλ(x)=11−x2+P V 1−1λ√π2√π√t−x dt=L(x)+(−1,1]v t(x)dM(t),where L(x):=1/(π√Vλ(x)(ι)has also positive lower bound a.e.on[a,b],assumingιis small enough. In addition,v t(x)≥0when t∈[0,x],whereas v t(x)≥B(x)≥−||B||[a,b]whent∈(x,1],so Vλ(x)(−)(ι)is bounded a.e.on[a,b].It follows that Vλ(x)(−)(ι)≤(1−η)Vλ(x)(+)(ι)a.e.x∈[a,b]for someη∈(0,1).Applying Lemma20we conclude that Vλ(x)(ι)has smooth integral on[a,b] (ifιis small enough).Therefore Vλ(x)has smooth integral by(44)and(45).We now restate Theorem4and prove it.Theorem24For a weight satisfying(19)and(20)we have Z¯R(W)=∅.That is,any continuous function g:¯R→R can be uniformly approximated by weighted polynomials W n p n(n=0,2,4,...)on¯R.Proof.Let x0∈¯R.We show that x0∈Z¯R(W).20First let us assume that x0isfinite.Choose J:=[a,b]such that a<x0<b holds.Let f(x)be a continuous function which is zero outside J and f(x0)=0. Let1<λ=u/v(u,v∈N+)be a rational number for which the conclusion of Lemma16holds.Now we use a powerful theorem of Totik.Since Vλhas a positive lower bound a.e.on J and it has smooth integral on J(see Lemma22), by[11],Theorem1.2,(a,b)∩Z(Wλ)=∅.So we canfind P n(n=0,1,2,...) such that(Wλ)n P n→f uniformly on¯R.So for n:=Nv,we haveW Nu p Nu→f,N=0,1,2,...,uniformly on¯R,(46) where p Nu:=P Nv and deg(p Nu)≤Nv≤Nu.For allfixed s∈{0,...,u−1}if we approximate f/W s instead of f at(46),it easily follows that there exist p k (k=0,1,2,...)such thatW k p k→f,k=0,1,2,...,uniformly on¯R.(47) Using only k=0,2,4,...,we get x0∈Z¯R(W)by Lemma23.Now let x0=∞.DefineW0(x):=1x).Note that1/W0(x)(=|x|/W(−1/x))and|x|/W0(−1/x)(=1/W(x))are posi-tive and convex functions because W satisfies(20)and(19).Let g be a continuous function on¯R.Define−1/∞to be0and−1/0to be∞.(So g(x)is continuous on¯R if and only if g(−1/x)is continuous on¯R.) Observe that for some p n we haveW n(x)p n(x)→g(x)(n=0,2,4,...)uniformly on¯R,iffW n(−1/x)p n(−1/x)→g(−1/x)(n=0,2,4,...)uniformly on¯R,iffW0n(x)q n(x),→g(−1/x)(n=0,2,4,...)uniformly on¯R,where q n(x):=x n p n(−1/x)are polynomials,deg q n≤n.Now let f(x)be a continuous function on¯R which is zero in a neighborhood of0but f(∞)=0.By what we have already proved,q n polynomials exist such that W0n(x)q n(x)(n=0,2,4,...)tends to f(−1/x)uniformly.Therefore we can approximate f(x)uniformly by W n(x)p n(x)(n=0,2,4,...),where p n(x):=x n q n(−1/x).Proof.Recall the definition:y(t)/x(t)=t,t∈¯R,where(x(t),y(t))∈Bd(K) and W(t):=|x(t)|.Definef(t):=f(x(t),y(t))=f(−x(t),−y(t)),t∈¯R.Note that if n is an even number(and a(n)kare unknowns)thennk=0a(n)kx n−k(t)y k(t)=x n(t)nk=0a(n)k y(t)Proof of Theorem3.Define f(x,y):=1,(x,y)∈Bd(K).By Lemma25there exist h2n∈H22n, n∈N,such that||1−h2n||Bd(K)→0.From here Theorem3follows the same way Theorem2follows from Lemma5.[7]E.B.Saff,Incomplete and orthogonal polynomials.In C.K.Chui,L.L Schu-maker,and J.D.Ward,editors,Approximation Theory IV,pp219-255.Academic Press,New York,1983[8]E.B.Saff,V.Totik,Logarithmic Potentials with External Fields,Springer-Verlag,Berlin,1997[9]P.Simeonov,A minimal weighted energy problem for a class of admissibleweights,Houston Journal of Mathematics,to appear[10]A.F.Timan,Theory of Functions of a Real Variable,(Moscow,1960)(inRussian).[11]V.Totik,Weighted polynomial approximation for convex externalfields,Constr.Approx.16(2)(2000)261-281.[12]V.Totik,Weighted approximation with varying weight.Lecture Notes inMathematics,1569.Springer-Verlag,Berlin,1994.[13]P.Varj´u,Approximation by homogeneous polynomials,submitted to Con-str.Approx.D.BenkoDepartment of MathematicsWestern Kentucky UniversityBowling Green,KY42101USAE-mail:dbenko2005@A.Kro´oAlfr´e d R´e nyi Institute of MathematicsHungarian Academy of SciencesH-1053Budapest,Re´a ltanoda u.13-15HungaryE-mail:kroo@renyi.hu23。
(5)Parallel spectral clustering in distributed systems

I. I NTRODUCTION Clustering is one of the most important subroutines in machine learning and data mining tasks. Recently, spectral clustering methods (e.g., [35] [31] [13]), which exploit pairwise similarities of data instances, have been shown to be more effective than traditional methods such as k -means, which only considers the similarity values from instances to k centers. (We denote k as the number of desired clusters.) Because of its effectiveness in finding clusters, spectral clustering has been widely used in several areas such as information retrieval and computer vision (e.g., [9] [49] [35] [50]). Unfortunately, when the number of data instances (denoted as n) is large, spectral clustering can encounter a quadratic resource bottleneck [13] [25] in computing pairwise similarity among n data instances, and in storing the large similarity matrix. Moreover, the algorithm requires considerable time and memory to find and store the first k eigenvectors of a Laplacian matrix. The most commonly used approach to address the computational and memory difficulties is to zero out some elements in the similarity matrix, or to sparsify the matrix. From the obtained sparse similarity matrix, one then finds
系统辨识综述

Contents lists available at ScienceDirect
Journal of Process Control
journal homepage: /locate/jprocont
b
a r t i c l e
i n f o
a b s t r a c t
Step and relay feedback tests have been widely used for model identification in the process industry. The corresponding identification methods developed in the past three decades are surveyed in this paper. Firstly, the process models with time delay mainly adopted for identification in the literature are presented with a classification on different response types. By categorizing the major technical routes developed in the existing references for parameter estimation relating to different applications, the identification methods are subsequently clustered into groups for overview, along with two specific categories for robust identification against load disturbance and the identification of multivariable or nonlinear processes. The rationales of each category are briefly explained, while a typical or state-of-the-art identification algorithm of each category is elucidated along with application to benchmark examples from the literature to illustrate the achievable accuracy and robustness, for the purpose of facilitating the readers to have a general knowledge of the research development. Finally, an outlook on the open issues regarding step or relay identification is provided to call attention to future exploration. © 2013 Elsevier Ltd. All rights reserved.
Visual Simulation of Smoke译文

虚拟烟雾模拟摘要:在这篇文章中,我们提出一种计算机图形应用领域中新的烟雾数值模拟的方法,即使用非粘性的欧拉方程作为烟雾的物理模型,这是一种稳定、快速并有很少的数值耗散的方法。
相比采用N-S方程的物理方法,它更加适合气体建模,并且计算量要小得多。
此外,我们还引入物理上一致的漩涡限制来保证烟雾在粗糙网格(相比使用更精细的计算流体力学文献中网格)上小尺度特征的模拟,并正确地处理与运动物体相互作用。
关键词:烟雾;计算流体动力学;Navier-Stokes方程;欧拉方程;半拉格朗日方法;稳定流体;漩涡限制;participating media参与媒体Ronald Fedkiw, Jos Stam and Henrik Wann Jensen, "Visual Simulation of Smoke", In SIGGRAPH 2001 Conference Proceedings, Annual Conference Series, August 2001, 15-221.引言:由于气体如烟雾的运动是极其复杂并难以捉摸的,所以模拟自然现象如烟雾等,在计算机图形领域仍然是一个具有挑战性的问题。
可视化的烟雾模型,广泛应用于包括特殊效果和互动游戏等相关行业领域,一个好的模型应该易于使用和生成并且具有很强的现实意义。
很显然,烟雾和气体的造型在其它工程领域也是非常重要的。
更普遍地讲,计算流体动力学广泛应用于气体和其他液体,例如水的模拟。
可直到最近,计算机图形学的研究人员才开始从大量的CFD文献中挖掘可以用于或修改计算机图形应用的方法。
不幸的是,目前的烟雾模型不是太缓慢,就是受到过多的数值耗散。
在本文中,我们采用CFD文献中有关气体如烟雾的动画仿真技术,提出了一种稳定、快速且没有过多数值耗散的模型,它使用了一种相对粗糙的网格,来生成复杂的滚动烟雾动画。
1.1 以往工作在过去的二十年里,烟雾及其它气体现象的建模,在计算机图形领域一直受到广泛关注。
液晶弹性常数测量+相延迟方法

Measurement of the twist elastic constant by phase retardation techniqueA. Nych, M. Voronin, V. Pergamenshchik,Yu. Kolomzarov*, V. Sorokin* and V. Nazarenko1Institute of Physics, 46 Nauki ave., Kyiv, 03039, Ukraine*Institute of Semiconductor Physics, 45 Nauki ave., Kyiv, 03028, UkraineWe have extended the experimental approach, used by Yu. A. Nastyshyn et al. [J. Appl. Phys. 86, 4199 (1999)] for measuring polar anchoring strength, to measure the twist elastic constant in nematic liquid crystal. The method implies measurements of phase retardation changes in liquid crystal under the action of magnetic field. The obtained experimental data were fitted with the predictions of elastic theory. We foundK, is in good agreement with those that the value of the measured elastic constant,22described in literature.Keywords: liquid crystal, elastic constant, phase retardation.IntroductionThe elastic constants of nematic liquid crystals have attracted considerable interest for many years and for numerous researchers [1–12]. This interest largely comes from the fact that elastic constants appreciably influence important display properties such as the threshold voltage, steepness of the electro-optical characteristic and so on. The demand to fabricate low power consumption, wide operation temperature range, high 1*****************.uacontrast and fast switching time LCDs provides the rationale for the precise knowledge of elastic constant values as well as their temperature dependence. The reason why many reports are still concerned with the elastic constants is that the measurements are far from to be trivial.The elastic constants of nematic liquid crystals are usually measured by the using Freedericksz transition induced by magnetic or electric field [1–6]. The experiments concern the investigation of direct measurement of the elastic torque exerted from a nematic sample on a glass plate were performed in [7, 10]. Other methods have been also proposed in [9] and in [11]. However, the most reliable values of elastic constants have been obtained from measurement of the Freedericksz transition in thin nematic layer. In most of these experiments the magnetic or electric field is applied perpendicular to the director. The director distortion occurs when the field exceeds a threshold value, C H . By assuming the strong anchoring of the director at the interface of the nematic layer one obtains aii C K d H χπ=, where d is the thickness of layer, a χ is anisotropy of magnetic susceptibility and ii K is an elastic constant (1=i – splay, 2=i – twist, 3=i – bend). The accuracy of the described method strongly depends on the director orientation at the interface. Even small deviation of the director results in sufficient shift of the threshold field.In the current paper we suggest an experimental technique to measure the twist elastic constant from the dependence of phase retardation of liquid crystal layer on the applied magnetic field. For a certain range of directions of the applied magnetic field the phase retardation demonstrates changes monotonically with the magnetic induction. 22K is determined from a simple fit of experimental curve.ExperimentExperiments were performed for the cells with alignment induced by coating of two glass substrates with the rubbed polyimide layer. The polyimide we used, LARC-CP1, provides almost planar orientation, the deviation of the angle between the substrate and director is less than o 1 [13]. The cells were filled with the nematic liquid crystal 5CB, purchased from EM Industries and used without any additional purification, with the parameters as following: extraordinary refractive index 708.1=e n[1], ordinary refractive index 530.1=o n [1], (both at wavelength nm 8.632=λ), anisotropy of magnetic susceptibility 3112105.113m kg a −−×=χ [5]. The optical phase retardation of the liquid crystal cell was determined by the Senarmont technique (see, for example [14, 15]). Schematically this setup is shown on Fig. 1. The light source was He-Ne laser (nm 633=λ). The linear polarized light entering the sample emerges elliptically polarized. Setting the optical axis of the quarter-wave plate parallel to the polarizer transforms the elliptically polarized light passed through the cell into linear polarized light. The measurement of the azimuth of this linear polarized light using the analyzer allows for determination of the phase retardation of the sample.Results and discussionA common nematic liquid crystal is uniaxial crystal, meaning that one crystal axis is different from the other two. The single crystal axis that is unique is often called the "extraordinary" axis and its associated refractive index is labeled e n , while the other two axes are "ordinary" axes with index o n [14]. The amount of phase retardation thatmonochromatic wave acquires from traveling trough a nematic liquid crystal is related to a refractive index, n , wavelength, λ, and the path length inside the crystal, L , as nL λπ=Φ2 (1) An input beam that is normally incident on the liquid crystal layer will be resolved into ordinary and extraordinary axis components, each with a different refractive index. The beam that emerges has a phase delay difference or retardation between the axes. Applied magnetic field results in reorientation of liquid crystal molecules along the direction of the field. Since the distortion starts in the middle of the cell the resultant structure consists of twist deformation with maximum amplitude in the center of the cell and the director coincides this rubbing directions at the interfaces. However, for normal incidence the polarization of light transmitted through the liquid crystal layer is not distinguishing from that transmitted through twisted nematic layer. There is no detectable retardation, since the polarization of light follows director deformation so the polarization of emerging light always corresponds to the director orientation at the interface. The light is "ignorant" of the deformation inside liquid crystal layer. But, when the cell is tilted toward or away from the light beam, the amount of retardation depends on the degree of tilt and has an angular sensitivity to director twist.Let us consider a homogeneously aligned nematic layer placed in magnetic field as it shown in Fig. 2. Input light, that is linearly polarized, makes a certain angle β with the cell. In the initial position the rubbing direction of the nematic cell was aligned along magnetic field. Then cell was rotated on an angle α around the normal to the cell in so manner that magnetic field induce only twist deformation of the director. In this geometry the effective extraordinary refractive index, eff n , of the LC becomesγ⋅+γ⋅⋅=2222cos sin e o oe eff n n n n n , (2)where γ is the angle between the director and input light beam. γ can be determined from defined angles β and α as follow ()ϕ−α⋅β−=γsin sin cos , where ϕ is the angle between director and rubbing direction. The angular dependent phase retardation of the liquid crystal layer is expressed as ∫−∆λπ=Φ222d d eff dz n , (3)where d is the thickness of liquid crystal layer and −γ⋅+γ⋅⋅β−=∆o e o o e i i eff n n n n n n n n 222222cos sin sin . (4)The first factor, β−22sin i in n , in eq. (4) originates from the increased optical pathlength and it takes into account a light refraction at the liquid crystal/glass interface. Just after passing an isotropic glass layer, ordinary and extraordinary beams will possess a different refraction indexes and thus they will propagate separately. To simplify our calculations we assume that both beams refract together with index of refraction, that equals to isotropic refractive index of the nematic liquid crystal, ()32e o i n n n +=.In the presence of the magnetic field, the Frank free energy density corresponding to the twist deformation is given by()202222121B n ⋅µχ− ∂ϕ∂=a d z K F (5)where 22K is the twist elastic constant, a χ is the anisotropy of the magnetic susceptibility, B is the magnetic field induction [16]. The magnetic field is taken to be small so that the energetic cost of surface director deviations can be neglected. In our geometry (see figure 2) we assume that angle α between B and rubbing direction, and thus the angle between nematic director n and B , is small. In this case equation (5) can be written as follow: ()()20222212121ϕ−α−µχ− ∂ϕ∂=B z K F a d , (6)where α is the angle between magnetic field B and rubbing direction (X -axis) and angle ϕ is the angle between nematic director n and rubbing direction. Solving the eq.(6) one can obtain the relationship between the angle of director deviation and the applied magnetic field as a function of z -coordinate:()()()()−α=ϕ2cosh cosh 1,d B q z B q B z , (6) where ()22022K B B q a µχ=, . Experimental data on the field induced change of the phase retardation, ()0=Φ−Φ=∆ΦB B B , allows one to compare these experimental data with the predictionof the elastic theory, eq. (6). The both experimental and theoretically calculated dependencies ()B ∆Φ are shown in Fig. 3, where the circles correspond to experimental values and solid lines represent calculated retardation. Within the all area of experimental data the theoretical fit is good. As an adjustable parameter to numerically solve the eq. (3) and (6) we used the elastic constant 22K . The best fit of experimentaldata provides us with the value of 22K . The measurements were carried out at the temperature 25 ºC.Thus, we obtained the following value of the twist elastic constant N 1013.31222−⋅=K . The estimated value of 22K elastic constant is approximately 10 % larger from those reported in [4] and is about the same order of magnitude with those reported in [9]. However, the choice of the a χ value may influence the value of the twist elastic constant measured in the present experiment.Finally we remark that we found good agreement of 22K with the values reported in literature [4, 9]. The developed approach can be very useful to measure the twist elastic constant in pretransitional phenomena like nematic to cholesteric transition or in twisted nematic. Further experimental and theoretical work is at present being carried out in order to make a comparison on the values of 22K obtained for uniformly aligned nematic layer and for twisted nematic layer.Acknowledgment.The authors thank Oleksandr Buluy and Andrey Dobrovolskii for help in developing of experimental set up. The present work was supported by STCU under grant #2025.Reference1. Karat P.P. and Madhusudana N.V.: Elastic and optical properties of some 4´-n-alkyl-4-cyanobiphenyls. Mol. Cryst. Liq. Cryst. 36, 51 (1976).2. Karat P.P. and Madhusudana N.V.: Elasticity and orientational order in some of4´-n-alkyl-4-cyanobiphenyls: Part II. Mol. Cryst. Liq. Cryst. 40, 239 (1977).3. Karat P.P. and Madhusudana N.V.: Orientational order and elastic constants ofsome cyanobiphenyls: Part III. Mol. Cryst. Liq. Cryst. 47, 21 (1978).4. Madhusudana N.V. and Pratibha R.: Elasticity and orientational order in some ofcyanobiphenyls: Part IV. Reanalisys of the data. Mol. Cryst. Liq. Cryst. 89, 249 (1982).5. Bradshow M.J. and Raynes E.P.: The Frank constants of some nematic liquidcrystals. J. Physique, 46, 1513 (1985).6. Schad Hp., Baur G. and Meier G.: Elastic constants and diamagnetic anisotropy ofp-disubstituted phelylcyclohexanes (PCH). J. Chem Phys., 70(06), 2770 (1979). 7. Faetti S., Gatti M. and Palleschi V.: Measurements of surface elastic torques inliquid crystals: a method to measure elastic constants and anchoring energies.Revue Phys. Appl. 21, 451 (1986).8. Coles H.J. and Sefton M.S.: Pretransitional behavior of the splay and twist elasticand viscotic constants for the nematic to smectic phase transition in octyl cyanobiphenyl (8CB). Mol. Cryst. Liq. Cryst. Lett. 4(5), 123 (1987).9. Breddels P.A. and Mulkens J.C.H.: The determination of the Frank elasticconstant for twist deformation of 4-n-pentyl-4´-cyanobiphenyl (5CB) using a conoscope. Mol. Cryst. Liq. Cryst. 147, 107 (1987).10. Faetti S. and Palleschi V.: The twist elastic constant and anchoring of the nematicliquid crystal 4-n-octyl-4´-cyanobiphenyl. Liquid Crystals, 2, 261 (1987).11. Bogi A. and Faetti S.: Elastic, dielectric and optical constants of 4´-pentyl-4-cyanobiphenyl. Liquid Crystals, 28, No. 5, 729 (2001).12. Scharkowski A., Crawford G.P., Zumer S. and Doane J.W.: A method for thedermination of the elastic constant ratio K33/K11in nematic liquid crystals.J. Appl. Phys., 73(11), 7280 (1993).13. Nazarenko V.G., Clair A.K.St., Klouda R., Polak R.D., Nastyshyn Yu. andLavrentovich O.D.: Chemically imidized polyiimide as an alignment material for LCDs. Journal of the SID, XXIX, 135 (1998).14. Born M. and Wolf E.: Principle of Optics. Pergamon, Oxford, 1980.15. Nastishin Yu.A., Polak R.D., Shiyanovskii S.V., Bodnar V.H. andLavrentovich O. D.: Nematic polar anchoring strength measured by electric field techniques. J. Appl. Phys. 86, 4199 (1999).16. de Gennes P.G.: The Physics of Liquid Crystals. Clarendon Press, Oxford, 1974.Figure captionsFigure 1. Experimental setup: L – laser, P – polarizer, R – rotating sample holder and LC cell, Q – quarter-lambda plate, A – Analyzer, Ph – Photodetector. The rotating sample holder allow the LC cell to be rotated around magnetic field direction B and around perpendicular to the cell surface direction (Z -axis). Rotation around the former axis changes the incidence angle of the laser beam β, while the angle α remains the same. Rotation around the Z -axis changes only the angle α. During these rotations magnetic field direction always lies in the plane of the cell.Figure 2. Illustration for theoretical calculations. The X -axis lies along the rubbing direction of the sample. Angle ϕ is the angle between nematic director n and X -axis (director deviation from rubbing direction). Angle α is the angle between magnetic field B and X -axis. PP’ is a line in XOY plane, perpendicular to the magnetic field direction B . Angle β is an incidence angle of laser beam. Note that during rotation around magnetic field direction only the β angle is changed and direction of light propagation K always lies in P ZP ′ plane. Angle γ is the angle between nematic director n and propagation direction of laser beam.Figure 3. Phase retardation as a function of the magnetic field. Lines represent theoretical fit for N 1013.31222−⋅=K of corresponding experimental data (squares, circles and triangles). The angle between magnetic field and rubbing direction o 20=α and the incident angle of laser beam for each data set is shown on the plot. Other parameters are as follows: 708.1=e n , 530.1=o n , 50=d µm, 3112105.113m kg a −−×=χ, 8.632=λ nm.Figure 1Figure 2Figure 3。
融合半变异函数的空间随机森林插值方法

DOI: 10.12357/cjea.20210628王铭鑫, 范超, 高秉博, 任周鹏, 李发东. 融合半变异函数的空间随机森林插值方法[J]. 中国生态农业学报 (中英文), 2022, 30(3): 451−457WANG M X, FAN C, GAO B B, REN Z P, LI F D. A spatial random forest interpolation method with semi-variogram[J]. Chinese Journal of Eco-Agriculture, 2022, 30(3): 451−457融合半变异函数的空间随机森林插值方法*王铭鑫1, 范 超2, 高秉博1**, 任周鹏3, 李发东3(1. 中国农业大学土地科学与技术学院 北京 100193; 2. 武汉大学遥感信息工程学院 武汉 430079; 3. 中国科学院地理科学与资源研究所 北京 100011)摘 要: 土壤环境变量具有较强空间异质性, 为空间插值精度的提升带来了困难, 仅基于空间相关性和空间异质性的空间插值方法难以获得较高的插值精度。
机器学习方法能够融合多维辅助变量的信息, 提高土壤属性的插值精度, 但是不能有效融合空间位置关系信息进一步改善插值精度。
本文基于随机森林空间预测框架, 将空间半变异函数与随机森林算法融合, 提出了融合半变异函数的空间随机森林插值方法。
应用所提出的方法对湖南省湘潭县土壤重金属数据进行空间插值, 并与随机森林方法、基于距离的随机森林空间预测方法、普通克里金方法和回归克里金方法进行对比, 检验了所提出方法的插值精度。
结果表明, 融合半变异函数的空间随机森林插值方法相较于传统克里金方法精度提升10%以上, 相较于新型机器学习空间插值方法精度提升5%以上, 同时基于半变异函数的空间随机森林插值方法的插值制图结果具有更加合理的空间分布和丰富的细节信息。
因此, 融合半变异函数的空间随机森林插值方法能够有效结合辅助变量信息与空间位置关系信息, 有效提高土壤环境变量插值精度。
描述一种研究方法英语作文

描述一种研究方法英语作文Research methods are essential tools for conducting scientific studies and acquiring new knowledge in various fields. These methods help researchers collect data, analyze information, and draw reliable conclusions. In this essay, we will explore a specific research method, namely experimental research, and discuss its processes, advantages, and limitations.Experimental ResearchExperimental research is a quantitative research method that involves manipulating variables and measuring their effects on other variables. The primary objective of this method is to establish cause-and-effect relationships between variables. It is often used in scientific studies, particularly in the fields of psychology, biology, and medicine. Processes of Experimental ResearchExperimental research typically follows several steps, including:1. Problem identification: Researchers identify a problem or research question to investigate. This step helps determine the purpose and objectives of the study.2. Literature review: Researchers review existing literature to gain a comprehensive understanding of the topic and previous studies. This step helps in formulating hypotheses and designing the experimental procedures.3. Formation of hypotheses: Based on the literature review, researchers formulate hypotheses that predict the relationship between variables. A hypothesis provides a clear direction for the experiment.4. Selection of participants: Researchers select a suitable sample of participants for the study. The sample should be representative of the target population to ensure the generalizability of the findings.5. Design of the experiment: Researchers design the experiment, including selecting the independent and dependent variables, determining the control group, and assigning participants to experimental and control conditions.6. Data collection: Researchers collect data through various methods, such as observation, surveys, interviews, or physiological measurements. The data collected should be valid and reliable.7. Data analysis: Researchers analyze the collected data using statistical techniques to test the hypotheses and determine if there is a significant effect of the manipulated variables.8. Interpretation of results: Based on the data analysis, researchers interpret the results and draw conclusions. They assess whether the experimental manipulation had an impact on the dependent variable and evaluate the significance of the findings.9. Reporting: Researchers write a research report or publish their findings in scientific journals. The report should include the research question,methodology, results, and conclusions.Advantages of Experimental ResearchExperimental research offers several advantages that contribute to its popularity:1. Control over variables: Experimental research allows researchers to control and manipulate variables, ensuring a cause-and-effect relationship can be established.2. Objectivity: The use of systematic procedures and data collection methods in experimental research promotes objectivity and reduces bias.3. Replication: Experimental research can be replicated by other researchers, which helps validate the findings and increase confidence in the results.4. Generalizability: With proper sampling techniques and study design, experimental research findings can be generalized to the target population.Limitations of Experimental ResearchDespite its advantages, experimental research also has limitations:1. Artificial settings: Experimental research often takes place in laboratory settings, which may not reflect real-world contexts accurately. This limitation raises concerns about the external validity of the findings.2. Ethical considerations: In some cases, manipulating variables in experimental research may raise ethical concerns, such as causing harmto participants or violating privacy.3. Time and resources: Conducting experimental research can betime-consuming and resource-intensive due to the need for precise control over variables and data collection.4. Generalizability limitations: The findings of experimental research may not always apply to real-world situations, as participants in laboratory experiments may behave differently from those in natural settings. ConclusionExperimental research is a valuable research method that allows researchers to establish cause-and-effect relationships between variables. It follows a systematic process and provides numerous advantages, such as control over variables and objectivity. However, it also has limitations, including artificial settings and potential ethical concerns. By understanding the processes, advantages, and limitations of experimental research, researchers can effectively utilize this method to advance scientific knowledge and contribute to their respective fields.。
A Simplified Evaluation OfStress Intensity Factor For A Small Diameter Pipe Penetrating A ThickPlate

c – half crack surface length a/c –aspect ratio a/t – normalized crack depth t – material thickness
INTRODUCTION
Recent discoveries of stress corrosion cracking in pressure vessel components has driven interest in developing better fracture mechanics based methods for determining remaining service life of reactor components [1]-[4]. Crack growth predictions are paramount to this goal and a stress intensity factor (SIF) history is an integral part of a crack growth model. Accuracy is of utmost importance because typical stress corrosion crack growth models amplify error in SIF history. The evaluation of a reactor component to obtain a SIF history can be cumbersome and a simplified method that proves to be accurate would greatly speed up the process.
Cornell University, Ithaca, NY
研究方法第一章讲义与习题

Chapter 1 Introduction1.1 Definitions of ‘research’1.1 Definition 1 of researchCharles and Mertler define it as “a careful, systematic, patient investigation undertaken to discover or establish facts and relationships.”1.1.2 Definition 2 of research.Another definition is offered by Hatch and Farhady, that is, research is …a systematic approach to finding answers to questions‟. …Hatch and Farhady‟s definition implicitly tells us three essential elements of research: questions, a systematic approach, and answers.Exercise 1.1To what extent do you believe the following activities involve a careful, systematic process?____ (1) Finding the year in which GXNU was established.____ (2) Preparing student achievement profiles for Guangxi‟s five largest universities.____ (3) Identifying the provincial group affiliations of 100 students randomly selected from College of Foreign Studies at GXNU and their relationship with cooperative learning.____ (4) Describing the daily English learning and practice activities of ten randomly selected students from College of Foreign Studies at GXNU.____ (5) Developing instructional activities that best promote English achievements in College of Foreign Studies at GXNU.1.2 Objectives of research1.2.1 DescriptionIt requires the researcher to portray the phenomenon accurately, to identify the variables that exist, and then determine the degree to which they exist.Any new area of study usually begins with the descriptive process because it identifies the variables that exist.1.2.2 ExplanationThis requires knowledge of why the phenomenon exists or what causes it. Of course most phenomena are multi-determined and that new evidence may necessitate replacing an old explanation with a better one.1.2.3 PredictionPrediction is the third objective of science, refers to the ability to anticipate an event prior to its actual occurrence.1.2.4 ControlControl refers to the manipulation of the conditions that determine a phenomenon. Once people in a certain field understand the conditions that produce a behavior, the behavior can be controlled by either allowing or notallowing the conditions to exist.Exercise:1. If you conducted a study in which you wanted to determine why help is not given to people who obviously need it, you would have conducted a study with which of the following objectives?A. DescriptionB. ExplanationC. PredictionD. Control1.3. Principles and rules of scientific research1.3.1. Legal principles:Rule 1. Protection.Rule 2. C onfidentiality. I1.3.2. Ethical principles.Rule 3. Beneficence.Rule 4. Honesty.Rule 5: Accurate Disclosure. TExercise for 1.2.2.1 – 1.2.2.2For each of the following, indicate the research operating rule(s) complied with or violated: (P)protection, (C)confidentiality, (B) beneficence, (H) honesty, or (AD) accurate disclosure__ __ 1. Jones‟s research assistant inadvertently mentioned the names of three high school students identified by Jones as from criminal families.____ 2. Jones noted poor test performance by a bright students. Realizing the performance did not reflect the student‟s ability, Jones changed the score to what he believed the student should have made.____ 3. Jones informed the students, thought not in detail, of the nature of the research in which they would be involved.____ 4. In the outdoor performance trials one of the participants succumbed to heat prostration中暑虚脱and had to be hospitalized overnight.____ 5. “A few cases seemed quite different from the rest, so we deleted them.”____ 6. “Requiring students to participate this activity might be harmful to some, but it is necessary for our research.”1.3.3. Philosophical principles.Rule 6: Importance.Rule 7: Generalizability.Rule 8: Replicability.Rule 9: Probability.1.3.4. Procedural principles.Rule 10: Researchability. TRule 11: Parsimony.Rule 12: CredibilityRule 13: Rival explanations forestalling.Exercise for 1.2.2.3-1.2.2.4Indicate which research principles have been observed or violated in the following: (S) significance, (G) generalizability, (RE) replicability, (PR) probability, (RS) researchability. (PS) parsimony, (C) credibility, or (RV) rival explanations__ 1. For his master‟s thesis in education, Allen wanted to study genealogical family roots in Italy.__ 2. Professor Douglas complimented Allen‟s revised research plan as one of the most concise and direct she had ever seen.__ 3. Norton wanted to repeat an earlier experiment on learning, but found that the documentation available was insufficient.__ 4. Professor Douglas told Norton that “The differences you found could as easily have been due to motivation as to intelligence.”__ 5. Norton wrote, “The data firmly prove the existence of a full year‟s difference in achievement.”___ 6. Professor Douglas determined that Norton‟s conclusions were not valid.1.4 General Classifications of Research1.4.1 Basic/theoretical Research, Applied Research and Practical research1.4.1.1 We need to construct theoretical models to explain general language acquisition, which can be categorized into basic or theoretical research.e.g.1Affective Filter hypothesise.g.2 The functioning of the right hemisphere of our brain is generally associated with holistic processing.1.4.1.2 We need to investigate the applications of theoretical constructs in linguistics and relevant fields of study to actual language teaching and learning contexts, which can be categorized into applied research.e.g.1. Most of the studies on Affective Filter hypothesis can be placed into one of the following three categories:(1) Motivation. Performers with high motivation generally do better in SLA.(2) Self-confidence. Performers with self-confidence and a good self-image tend to do better in SLA.(3) anxiety. Low anxiety appears to be condutice to SLA, whether measured as personal or classroom anxiety.e.g.2. Then an applied linguist who is interested in this theory makes an experiment to test to see whether students who are at the early stages of SLA are more likely to be characterized by heavy use of formulaic speech.1.4.1.3And we also need to utilize the theoretical and applied findings practically in language teaching methodologies, textbook compiling, and classroom language learning and observe their effects, which is practical research.Exercise for 1.3.1Place ‘B’ , ‘A’, ‘P ’ respectively in front of the examples of ‘basic’, ‘applied’ or‘practical’researc h.1. ____ As the phrase structure grammar can‟t deal with the ambiguity of the sentence “Flying planes can be dangerous”, the researcher tries to find another way todescribe this linguistic phenomenon among others.2. _____ To what extent a language laboratory could be used to teach spoken English.3. _____ The effectiveness of using manipulative materials in teaching fifth-grade English.1.4.2 Empirical (Primary) Research and non-empirical (Secondary) Research1.4.2.1Empirical research.Empirical research is research that bases its findings on direct or indirect observation as its test of reality. Such research may also be conducted according to hypothesis-deductive procedures.In practice, the accumulation of evidence for or against any particular theory involves planned research designs for the collection of empirical data, and academic rigor plays a large part of judging the merits of research design.1.4.2.2 Non-empirical researchPossible Interpretations of …Non-Empirical‟:(1) Not based on evidence from the real world; (2) Not based on new evidence from the real world (‘primary data’), but on data previously gathered, possibly for another, quite distinct purpose (…secondary data‟);(3) Risks being ivory-tower thinking, and producing results irrelevant to the real world.Exercise for 1.4.2Place an …E‟ in those that are examples of empirical research.1. ___ Logical inconsistencies in thesis writing of English postgraduates in China.2. ___ To what extent students‟ pragmatic knowledge is relate d to their foreign language proficiency.3. ___ The effects of cultural background knowledge on L2 reading comprehension.4. ___ A review on psycholinguistic research in China.5. ___ The effect of guided self-access English learning.6. ___ Sentences of same length but with different propositions are used to examine the effect of sentence complexity on the reading speed.1.4.3 Synthetic and Analytic research1.4.3.1 In synthetic or holistic approach, we attempt to grasp the whole or large parts of a multifaceted phenomenon in order to get a clearer idea of the possible interrelationships among the components.1.4.3.2 In analytic/constituent approach, we identify small parts of the whole for careful and close study, attempting to fit the small pieces into a coherent picture of the whole at a later state. It focuses on the role of the constituent parts that make up the total phenomenon.Exercise for 1.4.3Place an …A‟ in front of those that are examples of analytic research.1. ___ The perceptive comparison of stop consonants after initial /s/ in English words by English native speakers and Chinese learners of English.2. ___ The problems with the perception of continuous English speech at the speed of 130 wpm for Chinese learners of English..3. ___ How good learners and poor learners differ in reading strategies?4. ___ Does family income gap influence the effect of middle school English education in China?5. ___ The classroom behavior of English majors in the key university.1.4.4 Inductive research and Deductive researchAccording to the objective of a research study, we distinguish between heuristic and deductive research.1.4.4.1 Inductive researchThe inductive inquiry is a model in which general principles (theories) are developed from specific observations.1.4.4.2 Deductive researchIn deductive inquiry specific expectations of hypothesis are developed on1.4.5 Qualitative Research and Quantitative Research1.4.5.1 Quantitative researchQuantitative research is explaining phenomena by collecting numerical data that are analysed using mathematically based methods (in particular statistics).The process of measurement is central to quantitative research because it provides the fundamental connection between empirical observation and mathematical expression of quantitative relationships.Quantitative research is widely used in both the natural sciences and social sciences.1.4.5.2 Qualitative researchQualitative researchers aim to gather an in-depth understanding of human behavior and the reasons that govern such behavior. It is empirical research where the data are not in the form of numbers. It uses non-numerical data, so its data cannot be analyzed by using statistics.Of course this numeric –narrative contrast to capture their essential difference is oversimplified to some extent.I. Write …Q‟ if the characteristic refers primarily to one or more quantitative research methodologies.1. ___ A preference for hypotheses that emerge as the study progresses.2. ___ A preference for precise definitions stated at the outset of the study.3. ___ A preference for statistical summary of results.4. ___ Data are analysed inductively.5. ___ A preference for random techniques for obtaining meaningful samples.6. ___ A lot of attention devoted to assessing and improving the reliability of scores obtained from instruments.7. ___ A willingness to manipulate conditions when studying complex phenomena.8. ___ The researcher is the key instrument.9. ___ Primary reliance is on the researcher to deal with procedural bias.10. ___ the natural setting is the direct source of data.11. ___ Data are collected primarily in the form of numbers.II. Which of the following questions would lend themselves well to qualitative research?1. Do students learn more in a language laboratory than they do in a teacher-directed classroom?2. What sorts of conditioning drills do junior middle school English teachers use?3. How do elementary school English teachers teach children to read English materials?4. What kinds of things do Newly-graduated English teachers do as they go about their daily routine?5. How did teachers teach English during 1930s in China?6. What methods do the volunteer English tutors use in the after-school tutoring program?1.4.6 Experimental and non-experimental research1.4.6.1Experimental research can show cause-effect relationships morepersuasively than any other types of research. It is this knowledge of cause-effect which enables us to predict and control events. One essentail characteristics of it is that researchers manipulate one or more variables. r. 1.4.6.2 Nonexperimental research is generally conducted in a natural setting, with numerous variables operating simultaneously. Nonexperimental research is used to (1) depict people, events, situations, conditions and relationships as they currently exist or once exited; (2) evaluate products or processes; and (3) develop innovations.Exercise for 1.4.6Place an …E‟ in front of those that are exa mples of experimental research.1. ___ The influence of affective factors on the achievement of American literature.2. ___ Are cooperative learning approaches more effective than traditional whole-class learning approaches?3. ___ Will negotiated interaction between teacher and student facilitate foreign language comprehension?4. ___ To prove that deeper language processing provides a better memory of information, the researcher use three different questions to influence subjects‟ depth of processing.1.4.7 Longitudinal study and cross-sectional study1.4.7.1 Longitudinal study (纵向研究)The longitudinal study involves choosing a single group of participants and measuring them repeatedly at selected time intervals to note changes that occur over time in the specified characteristics.1.4.7.2 Cross-sectional researchThis type of study utilizes different groups of people who differ in the variable of interest, but share other characteristics such as socioeconomic status, educational background and ethnicity.Comparison between the two techniques:Cross-sectional research differs from a longitudinal research in that cross-sectional studies are designed to look at a variable at a particular point in time. While longitudinal studies involve taking multiple measures over an extended period of time,1.5 Common types of Research methodology1.5.1 SurveySurvey research a research method involving the use of questionnaires and/or statistical surveys to gather data about people and their thoughts and behaviours.1.5.2 Experimental and quasi-experimental researchExperimental research is the most conclusive of scientific methods. When doing an experiment we want to control the environment in a strict way so that we can find a definite causal relationship between variables we are concerned.Quasi-experimental designs are meant to approximate as closely as possible the advantages of true experimental designs. The main distinctionsbetween experimental and quasi-experimental research lie in the samplings and the size of controlling.Quasi-experimental research is especially suited to looking at the effects of an educational intervention. Its advantage over pure experimental designs is that they are studied in natural educational settings. This makes quasi-experimental research a good way of evaluating new initiatives and programmes in education.Exercise for 1.4.2Answer the following questions:1. What are the main differences between experimental and quasi-experimental studies?2. A researcher wants to know whether English teacher motivation improves student performance or whether it is higher student performance that motivates teachers. Is it possible to determine this? If yes, how would you do that?1.5.3 Historical researchHistorical research is the process of critical inquiry into past events to produce an accurate description and interpretation of those events. Careful consideration of their work can keep present-day educators from becoming lost.The sources of historical information are commonly classified as primary and secondary. A primary source is an original or firsthand account of the event or experience. A secondary source is an account that is at least once removed from the event.Exercise for 1.4.3Which of the following questions would lend themselves well to historical research? ( ) 1. What was life like for a woman English teacher in the 1980s?( ) 2. What sorts of techniques do English speech teachers use to improve an individual‟s ability to give an extemporaneous speech?( )3. What were the beginnings of the sociolinguistic studies?1.5.4 Case studyA case study is a study that examines one or more cases in detail by using multiple sources of data. the case study has a clear focus and the focused aspect should be examined within the context and has to be viewed as part of a system rather than as an isolated factor.In case study, the researcher collects the data from multiple sources by different techniques. Widely-used techniques include interviewing, think-aloud, diaries, etc.1.5.5 Ex post facto researchThe term “ex post facto” means from a thing done afterw ards; it implies some type of subsequent action. The variables are studied in retrospect, in search of possible relationships of effects.Exercise for 1.4.5A researcher is interested in the effects of location of school, grade level, and sex of the students on performance on a critical thinking test. A random sample is selected and measured using a published critical thinking test. Discuss why this is an example of ex post facto research rather than an experiment. Identify the independent and dependent variables.Answer: This is not an experiment, because there is no manipulation of independent variables. This study is ex post facto in nature, since the independent variables have already occurred and a retrospective search for cause-and-effect relationships is implied.Grade level, location of school, and sex of the student are independent variables. The dependent variable is critical thinking test performance.1.5.6 Action ResearchThe most quoted definition of action research is that of Carr and Kemmis: …a form of self-reflective enquiry undertaken by participants in social situations in order to improve the rationality and justice of their own practices, their understanding of these practices, and the situations in which the practices are carried out‟.Action research is a good way of professional development. It can help us to turn the problems we face in our professional careers into positive rather than negative experiences. Teacher education at the core is a process of reflection on professional action. It is this process that provides the momentum for increased professional competence.Exercise 1.4.6I. Which of the following questions might lend themselves well to action research?1. Do students learn more from older or younger teachers?2. Is the content found in our business English textbook biased, and if so, how?3. Would filmstrips help our College English teachers teach English syntax?4. Is phonics (看字读音教学法)more effective than look-say as a method of teaching English reading?5. What kinds of things do English Professors do as they go about their daily routine? II. In the space provided in front of each statement below, write …T‟ if the statement is true. Write …F‟ if the statement is false.1. ___ Action research is research conducted so that a decision can be reached about an issue of concern at the local school level.2. ___ Administrators would rarely participate in action research.3. ___ Those involved in action research generally want to solve some day-to-day immediate problem.4. ___ One advantage of practical action research is that it is not limited in generalizability.5. ___ An assumption underlying action research is that those who work in schools want to engage, at least to some degree, in some form of systematic research.6. ___ An important aspect of participatory action research is that the question or problem being investigated is one that is of interest to all the parties involved.7. ___ It is not unusual in action research to find the use of more than one instrument.8. ___Unfortunately, action research cannot help teachers to identify problems and issues systematically.1.5.7 Discourse analysisDiscourse analysis (DA) is a general term for a number of methods to analyzing written, spoken or signed language use.Discourse analysts study language use 'beyond the sentence boundary', as well as 'naturally occurring' language use, and not invented examples, which is known as corpus linguistics.1.5.8 Meta analysisMeta analysis is a quantitative approach that could be used to integrate and describe the results of a large number of studies.1.5.9 Correlational researchA correlational study consists of measuring two or more variables and then determining the degree of relationship that exists between them.1.5.10 Naturalistic observationNaturalistic observation as a research method involves observing subjects in their natural environment. This type of research is often utilized in situations where conducting lab research is unrealistic, cost prohibitive or would unduly affect the subject's behavior.1.5.11 Phenomenological researchThis type of method is used to descri be an individual’s, or group of individuals’, conscious experience of a phenomenon such as a counseling session, winning a speech contest.1.5.12Ethnographical researchEthnographical research can be used to describe and interpret the culture of a group of people. It has broad implications for many fields, including education. Professional development evaluators and staff developers can use this approach to understand teachers' needs, experiences, viewpoints, and goals.1.5.13 Comparative researchComparative research is a research methodology in the social sciences that aims to make comparisons across two or more things with a view to discovering something about one or all of the things being compared.1.5.14 Systematic reviewA systematic review is a literature review focused on a single question that tries to identify, appraise, select and synthesize all high quality research evidence relevant to that question.。
Crank–Nicolsonmethod
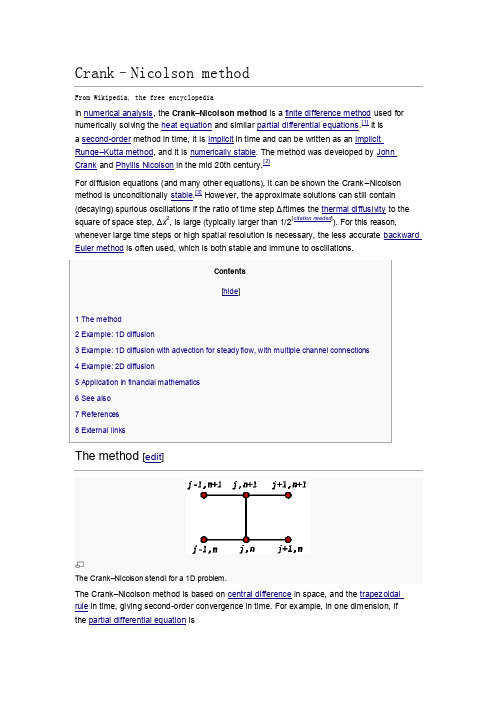
Crank–Nicolson method From Wikipedia, the free encyclopediathen, letting , the equation for Crank–Nicolson method is a combination of the forward Euler method at and the backward Euler method at n + 1 (note, however, that the method itself is not simply the average of those two methods, as the equation has an implicit dependence on the solution):The function F must be discretized spatially with a central difference.Note that this is an implicit method: to get the "next" value of u in time, asystem of algebraic equations must be solved. If the partial differentialequation is nonlinear, the discretization will also be nonlinear so thatadvancing in time will involve the solution of a system of nonlinear algebraicequations, though linearizations are possible. In many problems, especiallylinear diffusion, the algebraic problem is tridiagonal and may be efficientlysolved with the tridiagonal matrix algorithm, which gives a fast directsolution as opposed to the usual for a full matrix.The Crank–Nicolson method is often applied to diffusion problems. As anexample, for linear diffusion,whose Crank–Nicolson discretization is then:or, letting :which is a tridiagonal problem, so that may be efficientlysolved by using the tridiagonal matrix algorithm in favor of amuch more costly matrix inversion.A quasilinear equation, such as (this is a minimalistic exampleand not general)would lead to a nonlinear system of algebraic equationswhich could not be easily solved as above; however, it ispossible in some cases to linearize the problem by usingthe old value for , that is instead of .Other times, it may be possible toestimate using an explicit method and maintainstability.The Crank–Nicolson method is often applied to diffusion problems. As an example, for linear diffusion,whose Crank–Nicolson discretization is then:or, letting :which is a tridiagonal problem, so that may be efficiently solved by using the tridiagonal matrix algorithm in favor of a much more costly matrix inversion.A quasilinear equation, such as (this is a minimalistic example and not general)would lead to a nonlinear system of algebraic equations which could not beeasily solved as above; however, it is possible in some cases to linearize theproblem by using the old value for , that is instead of .Other times, it may be possible to estimate using an explicitmethod and maintain stability.。
科学文献

10 -17
0
50
100
150
200
250
Iteration number
Figure 1.1: SOR iteration where k k denotes any vector norm and the corresponding subordinate matrix norm. Rigal and Gaches 22] show that (y) is given by the explicit formula (y) = kAkkkrk+ kbk : (1.2) yk The forward error of y can be bounded using the standard perturbation result kx ? yk (A) (y) + O( (y) ); (1.3) kxk
Ax = b make the error and the residual in the presence of rounding errors? We
How small can a stationary iterative method for solving a linear system
Key words. stationary iteration, Jacobi method, Gauss-Seidel method, successive over-relaxation, error analysis, numerical stability. AMS subject classi cations.
b 0 1 b b b 238 b 13 b +2 b b 100
(y) = minf : (A + A)y = b + b; k Ak 2
扩散序谱(DOSY)实验中扩散系数维数字分辨率的影响

扩散序谱(DOSY)实验中扩散系数维数字分辨率的影响黄俊霖;余亦华【摘要】核磁共振二维扩散序谱(DOSY)实验测定溶液中分子自扩散系数时,扩散系数维的数据点及其数字分辨率直接影响了测定值的精度.在较系统地确定了DOSY 实验本身偏差范围的基础上,本文研究了扩散系数维不同的数字分辨率对测定值的影响,包括其引入偏差的来源以及形成偏差的大小.由于不同的溶液条件下分子扩散系数的改变可直接用于表征分子结构或状态的变化,本文提出的相对数字分辨率与扩散系数相对变化值的直接比较,可直观地表明数字分辨率对扩散系数测定值的影响.【期刊名称】《波谱学杂志》【年(卷),期】2018(035)003【总页数】7页(P287-293)【关键词】液体核磁共振(liquid-state NMR);数字分辨率;扩散序谱(DOSY);自扩散系数【作者】黄俊霖;余亦华【作者单位】华东师范大学物理与材料科学学院,上海市磁共振重点实验室,上海200062;华东师范大学物理与材料科学学院,上海市磁共振重点实验室,上海200062【正文语种】中文【中图分类】O482.53引言核磁共振扩散序谱(Diffusion Ordered Spectroscopy,DOSY)是目前测量液体样品的自扩散系数(D,简称扩散系数)的一个重要的方法,它通过脉冲梯度场(Pulsed Field Gradient,PFG)对溶液中分子的平移运动进行空间编码,在分子的扩散运动(扩散系数D)与梯度场强度g之间建立起明确的数学关系[1]:其中,I表示加上梯度场脉冲之后测得的信号强度;I0是未加梯度场脉冲时测得的信号强度;D为自扩散系数;γ为所观测核的磁旋比;g为梯度场强度;δ为梯度场脉冲宽度;Δ为脉冲序列中一对梯度场脉冲之间的时间,即扩散时间.将实验中不同梯度场强度下测得的谱峰强度 I代入(1)式,通过指数曲线的拟合便可求得D 值.一维谱中的谱峰产生于溶液中相同或不同的分子,通过谱峰积分面积随梯度场强变化拟合出的D值就是其对应的分子在该溶液体系中的扩散系数.与分别拟合出各个谱峰的D值不同,另一种呈现D拟合值的方式是二维DOSY谱[2].二维DOSY图谱中的其中一维是普通的化学位移轴,另一维则是扩散系数轴,相关峰所对应的扩散系数则是从该谱峰的最高点在扩散系数维的投影值(lgD)读出并换算而得,如图1所示.相关峰的产生首先是通过对其化学位移轴上相应数据点的峰强度变化拟合出,再对D值在一个预设的范围内进行反拉普拉斯变换后模拟生成的[2].虽然在一张DOSY谱上能够读取所有谱峰所对应的扩散系数,但是在DOSY图谱的数据处理时有2个人为设置的参数,即扩散系数的取值范围和数据点[3].扩散系数取值范围和数据点决定了该维读数的数字分辨率(数字分辨率 r=扩散系数的取值范围/数据点),而数字分辨率的大小直接影响到 DOSY实验中扩散系数读数的偏差. 图1 乙基苯样品的DOSY实验图谱(CDCl3)Fig. 1 DOSY spectrum of ethylbenzene (CDCl3)如图2所示,这是一张有关DOSY谱上扩散系数维的数据点及其数字分辨率r的示意图,纵坐标表示扩散系数维的取值,每一条虚线即代表该维的一个数据点,两条虚线间的间隔为该维的数字分辨率.当真实的谱峰最高点(lgD)出现在介于相邻的数据点之间时(如图 2中的椭圆形所示),由于数字分辨率的原因,图谱上实际的谱峰最高点会出现在其临近的数据点上(如图2上的矩形所示),因此图谱上扩散系数维设置的数字分辨率r(与扩散系数的取值范围和数据点相关)的设定影响了谱峰最高点读数的准确性,即为扩散系数维读数的最小偏差.当数字分辨率不够高时,有可能成为实验偏差的主要来源.图2 DOSY数字分辨率示意图Fig. 2 Diagram of digital resolution on F1 dimension (diffusional coefficient dimension) of DOSY spectrum从最初的自旋回波序列(Spin Echo,SE)序列[4]到后来的刺激回波(STimulated Echo,STE)序列[5]的衍变,以及纵向涡流延迟(Longitudinal Eddy current Delay,LED)技术[6]和偶极梯度场脉冲对(Bipolar Pulse Pairs,BPP)[7]技术的运用,大大提高了DOSY实验的可重复性和准确性,也大大拓展了DOSY实验的应用范围.DOSY谱中不同扩散速率分子产生的谱峰可以依据它们扩散的快慢沿着扩散系数维展开(谱峰不重叠的情况下),因此被广泛应用于复杂混合物的分析测试[8-10].更多的应用则是将溶液体系中扩散系数的变化用于表征分子间的相互作用[11,12],包括分子组装[13]、研究药物分子的包裹作用[14-17]以及聚合物分子量的测定等[18].在已发表的用扩散系数变化表征分子间相互作用的研究文献中,扩散系数的相对变化值通常在百分之几十~百分之十几之间[19-21],也有仅百分之几的变化[22],但提及扩散系数维的数据点数,以及考虑该维数字分辨率对研究结果的影响的研究并不多见.早期研究DOSY实验方法的文献曾经提及能观察到的扩散系数最小变化值在2%左右[20],而本文的研究结果表明如果数字分辨率运用得不合适,偏差会远大于 2%(见本文的研究结果与讨论),因此有必要对DOSY图谱扩散系数维数字分辨率的影响做一个较系统的研究,避免由此引起的实验偏差增加.数字分辨率的大小只是DOSY实验结果的偏差来源之一,它源于对实验数据的处理,而实验数据本身也会产生一个偏差范围.为了区分和比较两者的差别,本文首先研究了DOSY实验本身的重复性及偏差范围,继而分析研究了数字分辨率对扩散系数检测值的影响,阐述了实验偏差与数字分辨率的关系.1 实验部分1.1 仪器与测试样品测试仪器为Bruker公司的AvanceⅢ HD谱仪,配有BBFO 5 mm探头,1H核的共振频率为500.13 MHz.Z方向最大梯度场强度为53.5 Gauss/cm.测试样品为Bruker公司提供的标样,即0.1%乙基苯/氘代氯仿(EB/CDCl3)样品.1.2 NMR实验DOSY实验使用Bruker公司的标准脉冲序列stebpgp1s[4],谱宽为4 006.41 Hz,激发中心为2 250.59 Hz,H通道射频脉冲脉宽为10 μs,功率为21.8 W,弛豫延迟时间(D1)为2 s.累加次数为16,空采次数为16.脉冲序列中的扩散时间(Δ)为40 ms;梯度场脉冲宽度(δ)为1 800 μs,脉冲形状为梯形.每个DOSY实验中梯度场脉冲的强度(g)变化范围为5%~95%,采用线性方式在该范围中选取16个变化值.采样数据点为16 k,采样时间为2 s.1.3 数据处理所得的实验数据处理软件为Bruker公司提供的TopSpin 3.5pl7.通过其中的“DOSY”程序对实验得到的数据进行扩散系数拟合和反拉普拉斯变换,进而得到相应的DOSY图谱.处理过程中扩散系数维数据点为1 k,该维的取值范围(lgD)为-8 ~ -10,这两个处理参数其它的选值将在后面的讨论部分中加以具体说明.谱峰所对应的扩散系数值 D是由该峰的最高点在扩散系数维的投影值(lgD)读出并换算而得.2 结果与讨论2.1 DOSY实验偏差范围为了更准确地区分DOSY实验导致的偏差与数字分辨率引入的偏差,首先采用同样的样品与实验条件测试了6组DOSY实验以确定实验导致的偏差范围.每组实验连续测试5次,而1~6组实验依次之间的时间间隔为1天.图1为实验得到的DOSY图谱,其中以样品乙基苯中CH2峰(图中虚线所示位置)的数值代表乙基苯分子的扩散系数,共30个扩散系数值列于表1.表1 DOSY实验测得的乙基苯分子扩散系数Table 1 Self-diffusion coefficientsof ethylbenzene measured by DOSY experiments组别D/(×10-9 m2/s) 平均偏差d¯ 相对平均偏差d¯ r 1 1.611 1.618 1.611 1.611 1.625 0.0045 0.28%21.611 1.603 1.611 1.618 1.604 0.0047 0.29%3 1.596 1.604 1.618 1.611 1.5970.0074 0.46%4 1.582 1.575 1.575 1.582 1.582 0.0034 0.21%5 1.582 1.5751.582 1.582 1.589 0.0028 0.18%6 1.589 1.589 1.597 1.589 1.596 0.0036 0.23% 由于测试样品的溶剂为低粘度的氘代氯仿,有研究[23,24]表明这样的稀溶液在室温下只要存在控温的加热气流,就会产生沿NMR样品管方向的温度梯度,进而产生对流,影响扩散系数的测量.为避免此类现象对实验测量值造成偏差,上述所有实验都是在无温控和无温控气流的条件下进行的,实验室温度为(288±1)K.为了更好地呈现实验的偏差范围,采用了平均偏差()及相对平均偏差()的计算方法,其计算公式分别为:其中为扩散系数的平均值,n为计算所用的数据个数.表1中最后两列给出了每组5次连续实验的平均偏差()及相对平均偏差(),其相对平均偏差都在0.5%以下.将6组30个数值一起计算时,平均偏差为0.012 6;相对平均偏差为0.79%.数值都略大于同组内5个数据的偏差值,可能的原因可归于实验期间实验室温度的起伏以及仪器上不可预测的不稳定性.总体而言,实验本身的相对平均偏差约为 1%.为进一步确认此偏差范围,对上述30个实验中的CH2谱峰面积进行了积分,将积分值及其相应的实验参数代入(1)式进行D值的拟合(拟合程序为BRUKER公司提供的“T1/T2 measure”程序),拟合出的平均偏差为0.014 7,相对平均偏差为0.92%.与DOSY图谱中得到的数值偏差基本一致,即在控制了诸多的影响因素之后,得到的实验本身的相对偏差在1%左右. 2.2 关于DOSY数据处理中扩散维数字分辨率的问题上述DOSY图谱的处理中在扩散系数维的取值范围(lgD)在-8 ~ -10之间,该取值范围是有机化合物在溶液中常见范围[25],而该维所用的数据点是1 024,那么相邻两个数据点间lgD的差值为:[(- 8 )-(- 1 0)]/1024=0.00195,即数字分辨率(r)为0.001 95,对照表1可知其小于实验本身的平均偏差值,说明表1中DOSY实验数据偏差不完全是由数字分辨率的设置引入的.当扩散系数维是以对数形式(lgD)取值时,所有数据点间的间隔(即数字分辨率r)是相同的,而换算成 D值时,则数据点间隔是不等的.如图 2所示,x1数据点与相邻数据点间的间隔为(10 x 1 +r -10x1),而x2数据点与相邻数据点间的间隔则为( 1 0 x 2 + r -10x2),x1和x2分别为相应数据点对应的 lgD.由此可见直接用 D值表示时,数据点间隔与数据点所在位置对应的扩散系数值相关,相互之间是不等的.但是当引入相对数字分辨率(R=数据点间隔/数据点对应的D 值),则上述间隔值转换为: (1 0 x 1 + r -10x1)/10 x 1 = 1 0r -1,与x1、x2无关.这表明对于一个处理参数(取值范围和数据点)已确定的DOSY图谱,其相对数字分辨率( R = 1 0r -1)是一个恒定的值.正式发表的研究文献中通常都是直接用D值的变化大小,或以百分数表示其相对变化的大小,因此以相对数字分辨率R来表示其引入偏差的大小时更直观、更方便.根据上述所用的处理参数,图1中DOSY谱的数字分辨率r为0.001 95,相对数字分辨率R则为0.45%.为更明确地表明DOSY实验中数字分辨率的设置所引入的偏差,对表1中的第2组实验数据进行了不同数据点的处理,其结果列于表2.当取值范围(lgD)不变(-8 ~ -10),数据点为64和256时,它们所对应的相对数字分辨率R分别为7.46%和1.82%,表2中列出的5个数据之间只观察一个差值,其相对数据与相对数字分辨率基本一致,表明D值的偏差主要由数字分辨率不够高导致的.当数据点增加至1 024时,5个数据之间主要有2个差值(其中0.001的差值对于我们的研究没有意义,故舍去),其中一个的相对数据差值大于相对数字分辨率(约为2倍),说明此时的主要偏差不是来源于数字分辨率,因为数字分辨率引入的偏差只来源于相邻数据点的差别,不会大于相对数字分辨率本身.此外,由相对平均偏差的变化可知,随着数据点的增加,实验的精度也会逐渐提高.表2 不同数据点处理时扩散系数的偏差范围Table 2 Deviation range of self-diffusion coefficients when processing DOSY data with different data points*相对数据差=数据差值/数据点对应数值数据点D/(×10-9m2/s) 数据差值相对数据差* 相对数字分辨率相对平均偏差d¯r 64 1.778 1.655 1.778 1.778 1.778 0.123 7.43% 7.46% 3.94%256 1.625 1.625 1.625 1.655 1.655 0.030 1.85% 1.82% 1.44%1024 1.611 1.603 1.611 1.618 1.604 0.015 0.94% 0.45% 0.29%0.008 0.50%图3更直观地显示了数据点的多少对谱峰最高点(对应于扩散系数的读数)的影响,图中的谱峰来自于图1 DOSY图谱中虚线(通过CH2谱峰最高点)所在的化学位移位置(δ 2.67)对应的纵向一维谱.三角形、圆形和方形分别代表DOSY数据处理时所用数据点分别取128、512和1 024.从图中可以观察到当有足够多的数据点(如 1 024)时,这些数据点能较准确地描述出完整的峰型,包括谱峰的最高点;而数据点不足时不仅峰型描述得不够准确,更重要的是谱峰最高点的位置会随数据点移动,引起读数的偏差.图3 不同数据点处理时扩散系数维示意图Fig. 3 Sketch diagram of F1 dimension processed with different data points3 结论上述的研究结果表明DOSY图谱数据处理时如果设置的数据点不够多,导致数字分辨率不够高时,将会对实验所得的扩散系数测量值造成偏差,应当在设置相应的处理参数时予以充分考虑,并在成果发表时予以明确的陈述.当需要用扩散系数的变化值来表征分子结构或状态的变化时,相对数字分辨率应小于对应的相对变化值;当需要考虑扩散系数值的数据重复性时,建议相对数字分辨率R小于1%.从本文的研究结果中可知:扩散系数(lgD)取值范围为-8 ~ -10时,64、256和1 024个数据点对应的相对数字分辨率为7.46%、1.82%和0.45%.如果取值范围改变,则相对数字分辨率R= 1 0r -1(数字分辨率r=取值范围/数据点)也要做相应的调整.理论上如果相对数字分辨率为R,则由分辨率导致的读数偏差范围应该为(±R/2),由于数值范围上没有差别,为方便和容易理解,本文在所有的研究结果讨论中直接用R代替了±R/2.【相关文献】[1] JOHNSON C S. Diffusion ordered nuclear magnetic resonance spectroscopy: principles and applications[J]. ChemInform, 1999, 30(33):203-256.[2] LV J, SHAN L, TU G Z. The integrated DOSY acquisition/processing module for TopSpinNMR software[J]. Chinese J Magn Reson, 2008,25(1): 133-143.吕娟, 单璐, 涂光忠. TopSpin核磁共振软件中集成的DOSY采集/处理模块DOSYmTM[J]. 波谱学杂志, 2008, 25(1): 133-143.[3] ANTALEK B. Using pulsed gradient spin echo NMR for chemical mixture analysis: How to obtain optimum results[J]. Concept Magn Reson A,2010, 14(4): 225-258.[4] STEJSKAL E O, TANNER J E. Spin diffusion measurements: spin echoes in the presence of a time-dependent field gradient[J]. J Chem Phys,1965, 42(1): 288-292.[5] TANNER J E. Use of the stimulated echo in NMR diffusion studies[J]. J Chem Phys, 1970, 52(5): 2523-2526.[6] WU D H, CHEN A, JOHNSON C S. Flow imaging by means of 1D pulsed-field-gradient NMR with application to electroosmotic flow[J]. J Magn Reson, 1995, 115(1): 123-126. [7] WIDER G, DOTSCH V, WUTHRICH K. Self-compensating pulsed magnetic-field gradients for short recovery times[J]. J Magn Reson, 1994,108(2): 255-258.[8] BARJAT H, MORRIS G A, SMART S, et al. High-resolution diffusion-ordered 2D spectroscopy (HR-DOSY)-a new tool for the analysis of complex mixtures[J]. J Magn Reson, 1995, 108(2): 170-172.[9] Ca2+ assisted DOSY NMR: An unexpected tool for anomeric identification for D-glucopyranose[J]. ChemistrySelect, 2018, 3, DOI:10.1002/slct.201800316[10] TOUMI I, CALDARELLI S, TORRÉSANI B. A review of blind source s eparation in NMR spectroscopy[J]. Prog Nucl Magn Reson Spectrosc,2014, 81(8): 37-64.[11] PASTOR A, MARTÍNEZ-VIVIENTE E. NMR spectroscopy in coordination supramolecular chemistry: A unique and powerful methodology[J].Coordin Chem Rev, 2008, 252(21,22): 2314-2345.[12] MA E Q, LI Y X, ZHAO R G, et al. Interactions between NP-10 and single/double chain quaternary ammonium salts studied by NMR spectroscopy[J]. Chinese J Magn Reson, 2017, 34(1): 16-24.马二倩, 李永肖, 赵瑞格, 等. NP-10与单链、双链季铵盐三种复配体系相互作用规律的NMR研究[J]. 波谱学杂志, 2017, 34(1): 16-24.[13] KHODOV I A, ALPER G A, MAMARDASHVILI G M, et al. Hybrid multi-porphyrin supramolecular assemblies: Synthesis andstructure elucidation by 2D DOSY NMRstudies[J]. J Mol Struct, 2015, 1099: 174-180.[14] WANG L M, QIU R C, HUANG S H. Quantitative analysis of active ingredients in compound acetylsalicylic acid tablets by DOSY[J]. Chinese J Magn Reson, 2016, 33(3): 415-421.王丽敏, 仇汝臣, 黄少华. 复方乙酰水杨酸片中有效成分的DOSY技术分析[J]. 波谱学杂志, 2016, 33(3): 415-421.[15] TREFI S, GILARD V M M, MARTINO R. Generic ciprofloxacin tablets contain the stated amount of drug and different impurity profiles: A 19F,1H and DOSY NMR analysis[J]. J Pharmaceut Biomed Anal, 2007, 44(3): 743-754.[16] 崔艳芳, 刘买利. 应用DOSY-NMR分析血浆脂蛋白扩散系数的分布[C]. 南京: 第十一届全国波谱学学术会议, 2000.[17] ZHOU Q J, XIANG J F, TANG Y L. Applications of nuclear magnetic resonance spectroscopy in drug discovery[J]. Chinese J Magn Reson,2010, 27(1): 68-79.周秋菊, 向俊锋, 唐亚林. 核磁共振波谱在药物发现中的应用[J]. 波谱学杂志, 2010, 27(1): 68-79.[18] MONAKHOVA Y B, DIEHL B, DO T X, et al. Novel method for the determination of average molecular weight of natural polymers based on 2D DOSY NMR and chemometrics: Example of heparin[J]. J Pharm Biomed Anal, 2017, 149: 128-132.[19] BEDNAREK E, SITKOWSKI J, BOCIAN W, et al. An assessment of polydispersed species in unfractionated and low molecular weight heparins by diffusion ordered nuclear magnetic resonance spectroscopy method[J]. J Pharm Biomed Anal, 2010, 53(3): 302-308.[20] ANTALEK B. Using pulsed gradient spin echo NMR for chemical mixture analysis: How to obtain optimum results[J]. Concept Magn Reson A,2010, 14(4): 225-258.[21] ZHAO B, LI Y L, LI M, et al. An NMR study of capsaicin/β-cyclodextrin complex[J]. Chinese J Magn Reson, 2013, 30(4): 576-584.赵兵, 李艺蕾, 李明, 等. 辣椒碱与β-环糊精包合物的核磁共振研究[J]. 波谱学杂志, 2013, 30(4): 576-584.[22] CHEN X J, HU R Q, FENG H J, et al. Intradiffusion, density, and viscosity studies in binary liquid systems of acetylacetone + alkanols at 303.15 K[J]. J Chem Eng Data, 2012, 57(9): 2401-2408.[23] LOENING N M, KEELER J. Measurement of convection and temperature profiles in liquid samples[J]. J Magn Reson, 1999, 139(2): 334-341.[24] LAPPA M. Thermal convection: patterns, evolution and stability[M]. Wiley, 2009.[25] ANTALEK B. Using pulsed gradient spin echo NMR for chemical mixture analysis: How to obtain optimum results[J]. Concept Magn Reson A,2010, 14(4): 225-258.。
四氧化三铁磁性纳米微粒表面的氨基化修饰

第24卷第2期化学研究中国科技核心期刊2013年3月C H E M I C A L RESE ARC H hxyj@henu.edu.cn四氧化三铁磁性纳米微粒表面的氨基化修饰胡玮,娄兆文(湖北大学化学化T学院,湖北武汉430062)摘要:以3一氨丙基三乙氧基硅烷(APTES)作为氨基化试剂,通过硅烷化反应使其键合于Fe。
0。
纳米颗粒表面,制备了表面氨基化的磁性Fe。
O。
纳米复合颗粒;利用红外光谱分析了产物的化学键合特征,利用电位滴定测定了合成产物表面的一NH z含量,探讨了活化方式、反应溶剂、投料比、温度、时间等因素对氨基化修饰效果的影响.结果表明,APTES成功地包覆在磁性Fe。
0。
纳米微粒表面;在乙醇一水体系中,在Fe。
0。
与APTES投料比3:8、温度60℃下反应12 h,得到的Fe。
04纳米颗粒表面APTES修饰效果最佳,表面一NH:含量高达1 400±50/』mol·91。
.关键词:Fe。
O。
;磁性纳米颗粒;氨基化;表面修饰中图分类号:O 621文献标志码:A文章编号:1008—1011(2013)02—0144—05Amination surface-modification of ferriferous oxidemagnetic nanoparticlesH U Wei.LOU Zhao—we n。
(Coll ege of Chemis try and Che mica l Engineering,Hubei University,Wuhan 430062,Hubei,China)Abstract:3一Aminopropyl triethoxysilane(APTES)was used as the amination a g en t and coatedo n t o the surface of fer ri fer ou s oxi de(F e3 04)ma gnet ite n an opa rti cl es via silaniz ati o nrea cti on.The chemical bonding feature of as—synthesized APTES-coated Fe3 04 nanoparticles was a n a—lyzed by Four ie r trans fo rm in fra re d spectrometry,and its surface—NH2 c o n t e n t was determined by potentiometric titration technology.Furthermore,the influenc es of a ct iv ati o nmethod,sol—vent,fee ding r atio,rea ction temperature,and reaction ti me o n the modification effect of Fe3 04 nanopartic les b y—N H2 group we re investigated.Results show that Fe3 04nanoparticles have been successfully coate d by APTES.T he best su rf ace—mod if ic ati on effect isreached after Fe304 nanop articles a r e coated with A P T E S a t a feeding ratio of 3:8a nd atemperature of 60℃for12 h in the pr es e nc e of ethanol/water a s the solvent.Resultant surface-modified Fe3 04nanoparticles have a surface-NH2 c o n t e n t of a bo ut1400±50“m ol·g~.Keywords:ferriferous oxide(Fe304);magnetite nanoparticles;amination;surface modification纳米材料,特别是磁性纳米颗粒,是近年来物理、化学、化工、生物医药、材料科学与工程等领域研究的热点‘卜5|.国内外大量工作集中在磁性纳米颗粒的表面修饰.通过表面修饰使其功能化,应用于生物技术、医药和环境等众多领域邸].共混包埋法不需要进一步对微球表面进行化学处理就可以直接偶联所需的配体,是磁性纳米材料修饰极为有效的一种方法.目前普遍使用Fe。
Twisting Elements in Homotopy G-algebras

a rXiv:079.313v1[mat h.A T]2Se p27Twisting Elements in Homotopy G-algebras T.Kadeishvili Abstract We study the notion of twisting elements da =a ⌣1a with respect to ⌣1product when it is a part of homotopy Gerstenhaber algebra structure.This allows to bring to one context the two classical con-cepts,the theory of deformation of algebras of M.Gerstenhaber,and A (∞)-algebras of J.Stasheff.1Introduction A twisting element in a differential graded algebra (dga)(A ={A i },d :A n →A n +1,a m ·b n ∈A m +n )is defined as an element t ∈A 1satisfying the Brown’s condition dt =t ·t.(1)Denote the set of all twisting elements by T w (A ).An useful consequences of the Brown’condition is the following:let M be a dg module over A ,then a twisting element t ∈T w (A )defines on M a new differential d t :M →M by d t (x )=dx +t ·x ,and the condition (1)guarantees that d t d t =0.Twisting elements show up in various problems of algebraic topology and homological algebra.The first appearance was in homology theory offibre bundles [5]:For a fibre bundle F →E →B with structure group G there exists a twisting element t ∈A =C ∗(B,C ∗(G ))such that (M =C ∗(B )⊗C ∗(F ),d t )(the twisted tensor product)gives homology of the total space E .Later N.Berikashvili [4]has introduced in T w (A )an equivalence relation induced by the following group action.Let G be the group of invertible elements in A 0,then for g ∈G and t ∈T w (A )letg ∗t =g ·t ·g −1+dg ·g −1,(2)1easy to see that g∗t∈T w(A).The factor set D(A)=T w(A)/G,called Berikashvili’s functor D,has nice properties and useful applications.In par-ticular if t∼t′then(M,d t)and(M,d t′)are isomorphic.The notion of homotopy G-algebra(hGa in short)was introduced by Gerstenhaber and Voronov in[8]as an additional structure on a dg algebra (A,d,·)that induces a Gerstenhaber algebra structure on homology.The main example is Hochschild cochain complex of an algebra.Another point of view is that hGa is a particular case of B(∞)-algebra [10]:this is an additional structure on a dg algebra(A,d,·)that induces a dg bialgebra structure on the bar construction BA.There is the third aspect of hGa[16]:this is a structure which measures the noncommutativity of A.The Steenrod’s⌣1product which is the classical tool which measures the noncommutativity of a dg algebra(A,d,·)satisfies the conditiond(a⌣1b)=da⌣1b+a⌣1db+a·b+b·a.(3) The existence of such⌣1guarantees the commutativity of H(A),but a⌣1 product satisfying just the condition(3)is too poor for some applications. In many constructions some deeper properties of⌣1are needed,for example the compatibility with the dot product of A(the Hirsch formula)(a·b)⌣1c+a·(b⌣1c)+(a⌣1c)·b=0.(4) A hGa(A,d,·,{E1,k})is a dga(A,d,·)equipped additionally with a sequence of operations(some authors call them braces){E1,k:A⊗A⊗k→A,k=1,2,...}satisfying some coherence conditions(see bellow).The starting operation E1,1is a kind of⌣1product:it satisfies the conditions(3)and(4).As for the symmetric expressiona⌣1(b·c)+b·(a⌣1c)+(a⌣1b)·c,it is just homotopical to zero and the appropriate chain homotopy is the operation E1,2.So we can say that a hGa is a dga with a”good”⌣1product.There is one more aspect of hGa:the operation E1,1=⌣1is not associa-tive but the commutator[a,b]=a⌣1b−b⌣1a satisfies the Jacobi identity, so it forms on the desuspension s−1A a structure of dg Lie algebra.2Let us present three remarkable examples of homotopy G-algebras.Thefirst one is the cochain complex of1-reduced simplicial set C∗(X). The operations E1,k here are dual to cooperations defined by Baues in[2], and the starting operation E1,1is the classical Steenrod’s⌣1product.The second example is the Hochschild cochain complex C∗(U,U)of an associative algebra U.The operations E1,k here were defined in[14]with the purpose to describe A(∞)-algebras in terms of Hochschild cochains although the properties of those operations which where used as defining ones for the notion of homotopy G-algebra in[8]did not appear there.These operations where defined also in[10].Again the starting operation E1,1is the classical Gerstenhaber’s circle product which is sort of⌣1-product.The third example is the the cobar constructionΩC of a dg bialgebra C. The operations E1,k are constructed in[17].And again the starting operation E1,1is classical:it is Adams’s⌣1-product defined forΩC in[1]using the multiplication of C.The main task of this paper is to introduce the notion of a twisting element and their transformation in a hGa.Shortly a twisting element in a hGa(A,d,·,{E1,k})is an element a∈A such that da=a⌣1a and two twisting elements a,a=a+dg+g·g+g⌣1a+a;g,g)+E1,3(2[a,a].Butit is unclear wether the equivalence can be formulated in terms the bracket [,].In this paper we present the following application of the notion of twisting element in a hGa:it allows to unify two classical concepts,namely the the-ory of deformation of algebras of M.Gerstenhaber,and J.Stasheff’s A(∞)-algebras.Namely,a Gerstenhaber’s deformation of an associative algebra U(see [7],and bellow)a⋆b=a·b+B1(a⊗b)t+B2(a⊗b)t2+B3(a⊗b)t3+...∈U[[t]],can be considered as a twisting element B=B1+B2+...∈C2(U,U)in the Hochschild cochain complex of U with coefficients in itself:the defining3condition of deformation means exactly dB=B⌣1B.Furthermore,two deformations are equivalent if and only if the corresponding twisting elements are equivalent in the above sense.On the other side,the same concept of twisting elements in hGa works in the following problem.Suppose(H,µ:H⊗H→H)is a graded alge-bra.Let us define it’s Stasheff’s deformation as an A(∞)algebra structure (H,{m i})with m1=0and m2=µ,i.e.which extends the given algebra structure.Then each deformation can be considered as a twisting element m=m3+m4+...,m i∈C i(H,H)in the Hochschild cochain complex of H with coefficients in itself:the Stasheffs defining condition of A(∞)-algebra means exactly dm=m⌣1m.Furthermore,to isomorphic(as A(∞)-algebras)deformations correspond equivalent twisting elements in the above sense.In both cases we present the obstruction theory for the existence of suit-able deformations.The obstructions live in suitable Hochschild cohomologies: in H2(U,U)in Gerstenhaber’s deformation case and in H i(H,H),i=3,4,... in Stasheff’s deformation case.The structure of the paper is following.In the section2necessary def-initions are given.In the section3the definition Homotopy G-algebra is presented.In the section4the notion of twisted element in a homotopy G-algebra is studied.In the last two sections5and6the above mentioned applications of this notion are given.Acknowledgements.Dedicated to Murray Gerstenhaber’s80th and Jim Stasheff’s70th birthdays.2Notation and PreliminariesWe work over R=Z2.For a graded module M we denote by sM the suspension of M,i.e.(sM)i=M i−1.Respectively(s−1M)i=M i+1.2.1Differential Graded Algebras and CoalgebrasA differential graded algebra(dg algebra,or dga)is a graded R-module C={C i,i∈Z}with an associative multiplicationµ:C i⊗C j→C i+j and a differential d:C i→C i+1satisfying dd=0and the derivation condition4d(x·y)=dx·y+x·dy,where x·y=µ(x⊗y).A dga C is connected if C<0=0 and C0=R.A connected dga C is n-reduced if C i=0for1≤i≤n.A differential graded coalgebra(dg coalgebra,or dgc)is a graded R-module C={C i,i∈Z}with a coassociative comultiplication∆:C→C⊗C and a differential d:C i→C i+1satisfying dd=0and the coderivation condition∆d=(d⊗id+id⊗d)∆.A dgc C is connected if C<0=0and C0=R.A connected dgc C is n-reduced if C i=0for1≤i≤n.A differential graded bialgebra(dg bialgebra)(C,d,µ,∆)is a dg coalgebra (C,d,∆)with a morphism of dg coalgebrasµ:C⊗C→C turning(C,d,µ) into a dg algebra.2.2Cobar and Bar ConstructionsLet M be a graded R-module with M i≤0=0and let T(M)be the tensor algebra of M,i.e.T(M)=⊕∞i=0M⊗i.Tensor algebra T(M)is a free graded algebra generated by M:for a graded algebra A and a homomorphismα: M→A of degree zero there exists a unique morphism of graded algebras fα:T(M)→A(called multiplicative extension ofα)such that fα(a)=α(a). The map fαis given by fα(a1⊗...⊗a n)=α(a1)·...·α(a n).Dually,let T c(M)be the tensor coalgebra of M,i.e.T c(M)=⊕∞i=0M⊗i, and the comultiplication∇:T c(M)→T c(M)⊗T c(M)is given by∇(a1⊗...⊗a n)=nk=0(a1⊗...⊗a k)⊗(a k+1⊗...⊗a n).The tensor coalgebra(T c(M),∇)is a cofree graded coalgebra:for a graded coalgebra C and a homomorphismβ:C→M of degree zero there exists a unique morphism of graded coalgebras gβ:C→T c(M)(called comultiplica-tive coextension ofβ)such that p1gβ=β,here p1:T c(M)→M is the clear projection.The map gβis given bygβ=∞n=0(β⊗...⊗β)∆n,where∆n:C→C⊗n is n-th iteration of the diagonal∆:C→C⊗C,i.e.∆1=id,∆2=∆,∆n=(∆n−1⊗id)∆.Let(C,d C,∆)be a connected dg coalgebra and∆(c)=c⊗1R+1R⊗c+∆′(c).The(reduced)cobar constructionΩC on C is a dg algebra whose5underlying graded algebra is T(sC>0).An element(sc1⊗...⊗sc n)∈(sC)⊗n⊂T(sC>0)is denoted by[c1,...,c n]∈ΩC.The differential dΩofΩC for a generator[c]∈ΩC is defined by dΩ[c]=[d C(c)]+ [c′,c′′]where∆′(c)= c′⊗c′′,and is extended as a derivation.Let(A,d A,µ)be a1-reduced dg algebra.The(reduced)bar construction BA on A is a dg coalgebra whose underlying graded coalgebra is T c(s−1A>0). Again an element(s−1a1⊗...⊗s−1a n)∈(s−1A)⊗n⊂T c(s−1A>0)we denote as[a1,...,a n]∈BA.The differential d B of BA is defined byd B[a1,...,a n]= n i=1[a1,...,d A a i,...,a n]+ n−1i=1[a1,...,a i·a i+1,...,a n].2.3Twisting CochainsLet(C,d,∆)be a dgc and(A,d,µ)be a dga.A twisting cochain[5]is a homomorphismτ:C→A of degree+1satisfying the Brown’s conditiondτ+τd=τ⌣τ,(5) whereτ⌣τ′=µA(τ⊗τ′)∆.We denote by T w(C,A)the set of all twisting cochainsτ:C→A.There are universal twisting cochainsτC:C→ΩC andτA:BA→A being clear inclusion and projection respectively.Here are essential consequences of the condition(5):(i)The multiplicative extension fτ:ΩC→A is a map of dg algebras,so there is a bijection T w(C,A)↔Hom dg−Alg(ΩC,A);(ii)The comultiplicative coextension gτ:C→BA is a map of dg coalgebras, so there is a bijection T w(C,A)↔Hom dg−Coalg(C,BA).3Homotopy G-algebrasA homotopy G-algebra(hGa in short)is a dg algebra with”good”⌣1prod-uct.The general notion was introduced in[8],see also[24].Definition1A homotopy G-algebra is defined as a dg algebra(A,d,·)with a given sequence of operationsE1,k:A⊗(A⊗k)→A,k=0,1,2,3,...6(the value of the operation E1,k on a⊗b1⊗...⊗b k∈A⊗(A⊗...⊗A)we write as E1,k(a;b1,...,b k))which satisfies the conditionsE1,0=id,(6)dE1,k(a;b1,...,b k)+E1,k(da;b1,...,b k)+ i E1,k(a;b1,...,db i,...,b k)=b1·E1,k−1(a;b2,...,b k)+E1,k−1(a;b1,...,b k−1)·b k+i E1,k−1(a;b1,...,b i·b i+1,...,b k),(7)E1,k(a1·a2;b1,..,b k)=a1·E1,k(a2;b1,...,b k)+E1,k(a1;b1,...,b k)·a2+k−1p=1E1,p(a1;b1,...,b p)·E1,m−p(a2;b p+1,...,b k),(8) E1,n(E1,m(a;b1,...,b m);c1,...,c n)= 0≤i1≤j1≤...≤i m≤j m≤nE1,n−(j1+...+j m)+(i1+...+i m)+m (a;c1,...,c i1,E1,j1−i1(b1;c i1+1,...,c j1),c j1+1,...,c i2,E1,j2−i2(b2;c i2+1,...,c j2),c j2+1,...,c i m,E1,j m−i m(b m;c i m+1,...,c j m),c j m+1,...,c n).(9)Let us present these conditions in low dimensions.The condition(7)for k=1looks asdE1,1(a;b)+E1,1(da;b)+E1,1(a;db)=a·b+b·a.(10) So the operation E1,1is a sort of⌣1product:it is the chain homotopy which which measures the noncommutativity of A,c.f.the condition(3).Below we denote a⌣1b=E1,1(a;b).The condition(8)for k=1looks as(a·b)⌣1c+a·(b⌣1c)+(a⌣1c)·b=0,(11) this means,that the operation E1,1=⌣1satisfies the left Hirsch formula(4).The condition(7)for k=2looks asdE1,2(a;b,c)+E1,2(da;b,c)+E1,2(a;db,c)+E1,2(a;b,dc)=a⌣1(b·c)+(a⌣1b)·c+b·(a⌣1c),(12)this means,that this⌣1satisfies the right Hirsch formula just up to homo-topy and the appropriate homotopy is the operation E1,2.7The condition(9)for n=m=2looks as(a⌣1b)⌣1c+a⌣1(b⌣1c)=E1,2(a;b,c)+E1,2(a;c,b),(13) this means that the same operation E1,2measures also the deviation from the associativity of the operation E1,1=⌣1.3.1hGa as a B(∞)-algebraThe notion of B∞−algebra was introduced in[10]as an additional structure on a dg module(A,d)which turns the tensor coalgebra T c(s−1A)into a dg bialgebra.So it requires a differentiald:T c(s−1A)→T c(s−1A)which is a coderivation(that is an A(∞)-algebra structure on A,see bellow) and a an associative multiplicationµ:T c(s−1A)⊗T c(s−1A)→T c(s−1A)which is a map of dg coalgebras.Here we show that a hGa structure on A is a particular B(∞)-algebra structure:it induces on B(A)=(T c(s−1A),d B)a multiplication but does not change the differential d B(see[10],[16],[17],[18]for more details).Let us extend our sequence{E1,k,k=0,1,2,...}}to the sequence{E p,q: (A⊗p)⊗(A⊗q)→A,p,q=0,1,...}addingE0,1=id,E0,q>1=0,E1,0=id,E p>1,0=0,(14) and E p>1,q=0.This sequence defines a map E:B(A)⊗B(A)→A by E([a1,...,a m]⊗[b1,...,b n])=E p,q(a1,...,a m;b1,...,b n).The conditions(7)and(8)mean ex-actly dE+E(d B⊗id+id⊗d B)=E⌣E,i.e.E is a twisting cochain.Thus according to the section2.3it’s coextesionis a dg coalgebra mapµE:B(A)⊗B(A)→B(A).The condition(9)can be rewritten as E(µE⊗id−id⊗µE)=0,so this condition means that the multiplicationµE is associative.And the condition (14)means that[]∈B(A)is the unit for this multiplication.8Finally we obtained that(B(A),d B,∆,µE)is a dg bialgebra thus a hGa is a B(∞)-algebra.Let us mention,that a twisting cochain E satisfying just the starting condition(14)was constructed in[19]using acyclic models for A=C∗(X), the singular cochain complex of a topological space.The condition(14) determines this twisting cochain E uniquely up to equivalence of twisting cochains(2).3.2Homology of a hGa is a Gerstenhaber algebraA structure of a hGa on A induces on the homology H(A)a structure of Gerstenhaber algebra(G-algebra).Gerstenhaber algebra(see[6],[8],[24])is defined as a commutative graded algebra(H,·)together with a Lie bracket of degree-1[,]:H p⊗H q→H p+q−1(i.e.a graded Lie algebra structure on the desuspension s−1H)which is a biderivation:[a,b·c]=[a,b]·c+b·[a,c].Main example of Gerstenhaber algebra is Hochschild cohomology of an associative algebra.The following argument shows the existence of this structure on the ho-mology H(A)of a hGa.First,there appears on the desuspension s−1A a structure of dg Lie al-gebra:although the⌣1=E1,1is not associative,the condition(13)implies the pre-Jacobi identitya⌣1(b⌣1c)+(a⌣1b)⌣1c=a⌣1(c⌣1b)+(a⌣1c)⌣1b,this condition guarantees that the commutator[a,b]=a⌣1b+b⌣1a satisfies the Jacobi identity,besides the condition(10)implies that[,]: s−1A⊗s−1A→s−1A is a chain map.Consequently there appears on s−1H(A) a structure of graded Lie algebra.The Hirsch formulae(11)and(12)imply that the induced Lie bracket is a biderivation.3.3Operadic DescriptionThe operations E1,k forming hGa have nice description in terms of the sur-jection operad,see[20],[21],[3]for defily,to the dot product9corresponds the element(1,2)∈χ0(2);to E1,1=⌣1product corresponds (1,2,1)∈χ1(2),and generally to the operation E1,k corresponds the element E1,k=(1,2,1,3,...,1,k,1,k+1,1)∈χk(k+1).(15) We remark here that the defining conditions of a hGa(6),(7),(8),(9)can be expressed in terms of operadic structure(differential,symmetric group ac-tion and composition product)and the elements(15)satisfy these conditions already in the operadχ.Note that the elements(15)together with the element(1,2)generate the suboperad F2χwhich is equivalent to the little square operad([20],[21],[3]). This in particular implies that a cochain complex(A,d)is a hGa if and only if it is an algebra over the operad F2χ.This fact and the hGa structure on the Hochschild cochain complex C∗(U,U)of an algebra U[14]were used by some authors to prove the Deligne conjecture about the action of the little square operad on on the Hochschild cochain complex C∗(U,U).3.4Hochschild Cochain Complex as a hGaLet A be an algebra and M be a two sided module on A.The Hochschild cochain complex C∗(A;M)is defined as C n(A;M)=Hom(A⊗n,M)with differentialδ:C n−1(A;M)→C n(A;M)given byδf(a1⊗...⊗a n)=a1·f(a2⊗...⊗a n)+ n−1k=1f(a1⊗...⊗a k−1⊗a k·a k+1⊗..⊗a n)+f(a1⊗...⊗a n−1)·a n.We focus on the case M=A.In this case the Hochschild complex becomes a dg algebra with respect to the⌣product defined in[6]byf⌣g(a1⊗...⊗a n+m)=f(a1⊗...⊗a n)·g(a n+1⊗...⊗a n+m).In[14](see also[10],[8])there are defined the operationsE1,i:C n(A;A)⊗C n1(A;A)⊗...⊗C n i(A;A)→C n+n1+...+n i−i(A;A)10given by E 1,i (f ;g 1,...,g i )=0for i >n andE 1,i (f ;g 1,...,g i )(a 1⊗...⊗a n +n 1+...+n i −i )= k 1,...,k i f (a 1⊗...⊗a k 1⊗g 1(a k 1+1⊗...⊗a k 1+n 1)⊗a k 1+n 1+1⊗...⊗a k 2⊗g 2(a k 2+1⊗...⊗a k 2+n 2)⊗a k 2+n 2+1⊗...⊗a k i ⊗g i (a k i +1⊗...⊗a k i +n i )⊗a k i +n i +1⊗...⊗a n +n 1+...+n i −i ).(16)The straightforward verification shows that the collection {E 1,k }satisfies the conditions (6),(7),(8)and (9),thus it forms on the Hochschild complex C ∗(A ;A )a structure of homotopy G-algebra.We note that the operation E 1,1coincides with the circle product defined by Gerstenhaber in [6],note also that the operation E 1,2satisfying (12)and(13)also is defined there.4Twisting ElementsIn this section we present an analog of the notion of twisting element (see the introduction)in a homotopy G-algebra replacing in the defining equation da =a ·a the dot product by the ⌣1product.The appropriate notion of equivalence also will be introduced.Let (C ∗,∗,d,·,{E 1,k })be a bigraded homotopy G-algebra.That is (C ∗,∗,·)is a bigraded algebra C m,n ·C p,q ⊂C m +p,n +q ,and we require the existence of a differential (derivation)d (C m,n )⊂C m +1,n and of a sequence of operationsE 1,k :C m,n ⊗C p 1,q 1⊗...⊗C p k ,q k →C m +p 1+...+p k −k,n +q 1+...+q kso that the total complex (the total degree of C p,q is p +q )is a hGa.Bellow we introduce two versions of the notion of twisting elements in a bigraded homotopy G-algebra.Although it is possible to reduce them to each other by changing gradings,we prefer to consider them separately in order to emphasize different areas of their applications.The first one con-trols Stasheff’s A ∞-deformation of graded algebras and the second controls Gerstenhaber’s deformation of associative algebras,see the next two sections.114.1Twisting Elements in a Bigraded Homotopy G-algebra(version1)A twisting element in C∗,∗we define asm=m3+m4+...+m p+...,m p∈C p,2−psatisfying the condition dm=E1,1(m;m)or changing the notation dm= m⌣1m.This condition can be rewritten in terms of components asdm p=p−1i=3m i⌣1m p−i+2.(17)Particularly dm3=0,dm4=m3⌣1m3,dm5=m3⌣1m4+m4⌣1m3,.... The set of all twisting elements we denote by T w(C∗,∗).Consider the set G={g=g2+g3+...+g p+...;g p∈C p,1−p},and let us introduce on G the following operationg+g+∞k=1E1,k(g∗g)2=g∗g)3=g2⌣1g3;(g4+g3+g3⌣1g2+E1,2(m wherem;g,...,g),(19) particularlym4=m4+dg3+g2·g2+g2⌣1m3+m5=m5+dg4+g2·g3+g3·g2+g2⌣1m4+g3⌣1m3+m4⌣1g2+E1,2(Note that although in the right hand side of the formula(19)participatesm,thus this action is well defined:the components ofm is a twisting element.By D(C∗,∗) we denote the set of orbits T w(C∗,∗)/G.This group action allows us to perturb twisting elements in the following sense.Let g n∈C n,1−n be an arbitrary element,then for g=0+ 0g n+0+...the twisting elementm=m3+...+m n+(m n+1+dg n)+m n+3+...,(20) so the components m3,...,m n remain unchanged andm=m3+m4+...+m p+...thefirst component m3is a cycle and by(19) each perturbation of m leaves the class[m3]∈H3,−1(C∗,∗)unchanged and this class is thefirst obstruction for triviality.If this class is zero,then we choose g2∈C2,−1such that dg2=m3.Perturbing m by g=g2+0+0+... we kill thefirst component m3,i.e.we get the twisting elementm=0+m5+....Now,since of(17),the componentm4.If it is not then we take another g2 and try new second obstruction.Generally after killingfirst components,for m=0+0+...+0+m n+m n+1+...the obstruction is the homology class [m n]∈H n,2−n(C∗,∗).This in particular implies that if for a bigraded homotopy G-algebra C∗,∗all homology modules H n,2−n(C∗,∗)are trivial for n≥3,then D(C∗,∗)=0, thus C∗,∗is rigid.4.2Twisting Elements in a Bigraded Homotopy G-algebra(version2)This version can be obtained from the previous one by changing grading: take new bigraded module C∗,∗into a bigraded hGa.A twisting element m∈C∗,2−∗in this case looks as b=b1+b2+...+ b n+...,b n∈C∗,∗)by the rule g∗b=b′whereb′=b+dg+g·g+E1,1(g;b)+∞k=1E1,k(b′;g,...,g).(21)By D′(C∗,∗)/G′.We consider the following two problems.Quantization.Thefirst component b1∈C∗,∗).Thus we have a correct mapψ:D′(C∗,∗).14A quantization of a homology classα∈H2,1(C∗,∗),n≥2.Rigidity.A twisting element b=b1+b2+...is called trivial if it is equivalent to0.A bigraded hGaC∗,∗)={0}.The obstructions to triviality of a twisting element lay in homologies H2,n(C∗,∗we have H2,n(C∗,∗)=0thus2[m,m],sothe same m can be regarded as a Lie twisting element in the bigraded dg Lie algebra L∗,∗.So the notion of the twisting element in a hGa,which involves just the operation E1,1=⌣1in fact can be expressed in terms of Lie bracket[,].But it is unclear whether the group action formulas(19)and(21),which involve all the operations{E1,k,k=1,2,...}can be expressed just in terms of bracket.5Deformation of AlgebrasThis is just illustrative ing the homotopy G-algebra structure, the notions of twisting element and their transformation one can obtain the well known results of Gerstenhaber from[7].Let(A,·)be an algebra over afield k,k[[t]]be the algebra of formal power series in variable t and A[[t]]=A⊗k[[t]]be the algebra of formal power series with coefficients from A.15Gerstenhaber’s deformation of an algebra(A,·)is defined as a sequence of homomorphismsB i:A⊗A→A,i=0,1,2,...;B0(a⊗b)=a·bsatisfying the associativity conditioni+j=n B i(a⊗B j(b⊗c))=i+j=nB i(B j(a⊗b)⊗c).(22)Such a sequence determines the star producta⋆b=a·b+B1(a⊗b)t+B2(a⊗b)t2+B3(a⊗b)t3+...∈A[[t]], which can be naturally extended to a k[[t]]−bilinear product⋆:A[[t]]⊗A[[t]]→A[[t]]and the condition(22)guarantees it’s associativity.Two deformations{B i}and{B′i}are called equivalent if there exists a sequence of homomorphisms{G i:A→A;i=0,1,2,...;G0=id}suchthatr+s=n G r(B s(a⊗b))=i+j+k=nB′i(G j(a)⊗G k(b)).(23)The sequence{G i}determines homomorphism G= G i t i:A→A[[t]].On it’s turn this G naturally extends to a k[[t]]−linear bijection(A[[t]],⋆)→(A[[t]],⋆′)and the condition(23)guarantees that this extension is multi-plicative.A deformation{B i}is called trivial,if{B i}is equivalent to{B0,0,0,...}. An algebra A is called rigid,if each it’s deformation is trivial.Now we present the interpretation of deformations and their equivalence in terms of twisting elements of version2type and their equivalence in hGa of Hochschild cochains.As it is mentioned in3.4the Hochschild complex C∗(A,A)for an algebra A is a homotopy G-algebra.Then the tensor product C∗,∗=C∗(A,A)⊗k[[t]] is a bigraded Hirsch algebra with the following structureC p,q=C p(A,A)·t q,δ(f·t q)=δf·t q,f·t p⌣g·t q=(f⌣g)·t p+q,E1,k(f·t p;g1·t q1,...,g k·t q k)=E1,k(f;g1,...,g k)·t p+q1+...+q k,here we use the notation f⊗t p=f·t p.16Then each deformation{B i:A⊗2→A,i=1,2,3,...}can be interpreted as a version2type twisting element b=b1+b2+...+b k+...,b k=B k·t k∈C2,k: the associativity condition(22)can be rewritten asB i·t i⌣1B j·t j.δB n·t n=i+j=nSuppose now two deformations{B i}and{B′i}are equivalent,i.e.there exists{G i}such that the condition(23)satisfied.In terms of Hochschild cochsins this condition looks asb′=b+δg+g⌣g+g⌣1b+E1,1(b′;g)+E1,2(b′;g,g),where g=g1+...+g k+...,g k=G k·t k∈C1,k.This equality slightly differs from(21),but since g∈C1(A,A)and b′∈C2(A,A)),we have E1,k(b′;g,...,g)=0for k≥3(see3.4),thus they in fact coincide.So we obtain that deformations are equivalent if and only if the corre-sponding Hochschild twisting elements b and b′are equivalent.Consequently the set of equivalence classes of deformations is bijective to D′(C∗,∗).It is clear that H p,q(C∗,∗)=HH p(A,A)·t q.Then from the section4.2 follow the classical results of Gerstenhaber:obstructions for quantization of a homomorphism b1:A⊗A→A lay in HH3(A,A),and if HH3(A,A)=0 then each b1is quantizable(or integrable as it is called in[7]).Furthermore, the obstructions for triviality of a deformation lay in HH2(A,A),and if HH2(A,A)=0then A is rigid.Remark1As we see in the definition of equivalence of deformations par-ticipate just the operations E1,1and E1,2,the higher operations E1,k,k>2 disappear since of(16).So observing just deformation problem it is impossi-ble to establish general formula(21)for transformation of twisting elements.6A(∞)-deformation of Graded AlgebrasIn this section we give the similar description of A(∞)-deformation of graded algebras in terms of twisting elements in the hGa of Hochschild cochains.So this two types of deformation will be unified by the notion of twisting element in hGa.Partially these results are given in[14],[15].176.1A(∞)-algebrasThe notion of A(∞)-algebra was introduced by J.D.Stasheffin[23].This notion generalizes the notion of dg algebra.An A(∞)-algebra is a graded module M with a given sequence of opera-tions{m i:M⊗i→M,i=1,2,...,deg m i=2−i}which satisfies the following conditionsi+j=n+1n−jk=0m i(a1⊗...⊗a k⊗m j(a k+1⊗...⊗a k+j)⊗...⊗a n)=0.(24)Particularly,for the operation m1:M→M we have deg m1=1and m1m1=0,this m1can be regarded as a differential on M.The operation m2:M⊗M→M is of degree0and satisfiesm1m2(a1⊗a2)+m2(m1a1⊗a2)+m2(a1⊗m1a2)=0,i.e.m2can be regarded as a multiplication on M and m1is a derivation. Thus(M,m1,m2)is a sort of(maybe nonassociative)dg algebra.For the operation m3we have deg m3=−1andm1m3(a1⊗a2⊗a3)+m3(m1a1⊗a2⊗a3)+m3(a1⊗m1a2⊗a3)+m3(a1⊗a2⊗m1a3)+m2(m2(a1⊗a2)⊗a3)+m2(a1⊗m2(a2⊗a3))=0, thus the multiplication m2is homotopy associative and the appropriate chain homotopy is m3.The sequence of operations{m i}determines on the tensor coalgebraT c(s−1M)=R+s−1M+s−1M⊗s−1M+s−1M⊗s−1M⊗s−1M+...a coderivationd m(a1⊗...⊗a n)=k,ja1⊗...⊗a k⊗m j(a k+1⊗...⊗a k+j)⊗...⊗a n,and the condition(24)is equivalent to d m d m=0.The obtained dg coalgebra (T c(s−1M),d m)is called bar construction and is denoted as B(M,{m i}).18A morphism of A (∞)-algebras (M,{m i })→(M ′,{m ′i })is defined as a sequence of homomorphisms{f i :M ⊗i →M ′,i =1,2,...,deg f i =1−i },which satisfy the following conditioni +j =n +1 n −j k =0f i (a 1⊗...⊗a k ⊗m j (a k +1⊗...⊗a k +j )⊗...⊗a n )= k 1+...+k t =n m ′t (f k 1(a 1⊗...⊗a k 1)⊗f k 2(a k 1+1⊗...⊗a k 1+k 2)⊗...⊗f k t (a k 1+...+k t −1+1⊗...⊗a n )).(25)In particular for n =1this condition gives f 1m 1(a )=m ′1f 1(a ),i.e.f 1:(M,m 1)→(M ′,m ′1)is a chain map;for n =2it givesf 1m 2(a 1⊗a 2)+m ′2(f 1(a 1)⊗f 1(a 2))=m ′1f 2(a 1⊗a 2)+f 2(m 1a 1⊗a 2)+f 2(a 1⊗m 1a 2),thus f 1:(M,m 1,m 2)→(M ′,m ′1,m ′2)is multiplicative up to homotopy f 2.A collection {f i }defines a homomorphism f :B (M,{m 1})→M ′.It’s comultiplicative coextension ,see 2.2,is a graded coalgebra map of the bar constructionsB (f ):B (M,{m i })→B (M ′,{m ′i }),and the condition (25)guarantees that B (f )is a cahin map,i.e.B (f )is a morphism of dg coalgebras.So B is a functor from the category of A (∞)-algebras to the category of dg coalgebras.A weak equivalence of A (∞)-algebras is defined as a morphism f ={f i }for whichB (f )is a weak equivalence of dg coalgebras.It is possible to show (see for example [15])that(i)f is a weak equivalence of A (∞)-algebras if and only if f 1is a weak equivalence of dg modules;(ii)f is an isomorphism of A (∞)-algebras if and only if f 1is an isomorphism of dg modules.An A (∞)-algebra (M,{m i })we call minimal if m 1=0.In this case (M,m 2)is strictly associative graded algebra.The following proposition is the imediate consequence of (i)and (ii):Proposition 1Each weak equivalence of minimal A (∞)-algebras is an iso-morphism.19。
介绍研究方法英语作文

介绍研究方法英语作文介绍研究方法英语作文 Embarking on a research journey, whether in the sprawling domains of science, the intricate tapestry of social sciences, or the boundless realm of humanities, demands a meticulous selection of research methods. These methods act as our guiding stars, illuminating the path towards credible and insightful conclusions. Much like a skilled craftsman selects the perfect tool for each task, a researcher must carefully choose the methods that best suit theunique contours of their research question. Quantitative methods, the championsof numerical data and statistical analysis, offer a robust framework for investigating the "what" and "how much" of a phenomenon. Surveys, with their sweeping reach, allow us to gather data from a large population, painting a broad picture of trends and patterns. Experiments, on the other hand, delve deeper into cause-and-effect relationships, meticulously controlling variables to isolate the impact of specific factors. These methods, with their emphasis on objectivity and generalizability, form the bedrock of scientific inquiry. Yet, the human experience is not solely composed of quantifiable elements. To truly understandthe "why" and "how" behind human behavior and social phenomena, we turn to qualitative methods. Interviews, with their in-depth explorations, allow us to delve into the thoughts, feelings, and motivations of individuals. Observations, with their immersive quality, enable us to witness social interactions andcultural nuances firsthand. These methods, with their emphasis on subjectivity and context, provide rich, nuanced insights into the human experience. The choice between these methodological approaches is not always a clear-cut dichotomy. Often, researchers employ a mixed-methods approach, weaving together the strengths ofboth quantitative and qualitative methods. This allows for a more comprehensive understanding of the research problem, encompassing both the statistical trendsand the lived experiences of individuals. For instance, a study on the impact of social media on mental health might utilize surveys to gauge the prevalence of anxiety and depression among users, while also conducting interviews to explorethe personal narratives and coping mechanisms of individuals who have experienced these challenges. The selection of research methods is further influenced by the nature of the research question. Exploratory research, venturing into unchartedterritory, often relies on qualitative methods to generate hypotheses and identify key variables. Descriptive research, aiming to paint a detailed picture of a phenomenon, might employ surveys and observational studies to gather rich, descriptive data. Explanatory research, seeking to unravel the causal mechanisms underlying a phenomenon, often turns to experiments and quantitative analysis to establish clear cause-and-effect relationships. In conclusion, the choice of research methods is a pivotal decision that shapes the entire research process. By carefully considering the research question, the desired level of depth, and the nature of the data, researchers can select the methods best suited to illuminate the path towards meaningful and impactful conclusions. Whether embracing the rigor of quantitative analysis or delving into the richness of qualitative exploration, the chosen methods act as our compass, guiding us towards a deeper understanding of the world around us.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2
Twisted factorization
Let T be an n-by-n complex symmetric tridiagonal matrix, then P = T T H is Hermitian pentadiagonal. Suppose that we have the LDLH decomposition P = LD LH , where 1 ˆ l1 ˆ1 , ..., d ˆn ), d ˆi ≥ 0, and L = D = diag(d m ˆ1 0
Key words: twisted factorization, symmetric SVD, Takagi factorization. AMS subject classifications(1991): 15A18, 65F20, 65F25, 65F50.
1
Introduction
Complex symmetric matrices arise from many applications, such as chemical exchange in nuclear magnetic resonance and power systems. Singular value decomposition (SVD) reveals a great deal of properties of a matrix. A complex symmetric matrix has a symmetric SVD (SSVD), also called Takagi factorization, which exploits the symmetry [1]. Let A be a complex symmetric matrix, its Takagi factorization has the form: A = U ΣU T , where U is unitary and Σ the diagonal singular value matrix. The columns of U are called the Takagi vectors. As the case of a general matrix, computing the Takagi factorization of a complex symmetric matrix consists of two stages: tridiagonalization and diagonalization. In the first stage, a complex symmetric matrix A is reduced to a complex symmetric tridiagonal T
,
. . . .. .. .
0 . . mn−2 ln−1 1..DL = diag(α1 , ..., αn ), αi ∈ R,
and
It is called twisted factorization because Nk consists of part of the lower triangular L and part of the upper triangular U in (1). In the next section, based on the twisted factorization, we will show how to efficiently compute the eigenvectors of P , which are the left singular vectors of T . To obtain the decomposition P − µI = LDL LH , we compare the entries on the both ˆi and sides of LDLH − µI = LDL LH . Specifically, the (i + 2, i)-entry of LD LH − µI is m ˆ id ˆi /αi . The (i + 2, i + 1)the corresponding entry of LDL LH is mi αi . Thus, we have mi = m ˆ id ∗ ∗ ˆ ˆ ˆ ˆ entries of the both sides are m ˆ i li di + li+1 di+1 and mi li αi + li+1 αi+1 , where x∗ denotes ˆi + |ˆ ˆi+1 + d ˆi+2 − µ the complex conjugate of x. The (i + 2, i + 2)-entries are |m ˆ i |2 d li+1 |2 d 2 2 and |mi | αi + |li+1 | αi+1 + αi+2 . In summary, we have the following algorithm for the decomposition P − µI = LDL LH . Algorithm 2.1 Given the LDLH decomposition P = LDLH of a Hermitian pentadiagonal P = T T H , it computes the LDLH decomposition of the shifted P − µI = LDL LH . ˆ1 − µ; α1 = d % (1, 1)-entry ˆ1 /α1 ; l1 = ˆ l1 d % (2, 1)-entry 2 2 ˆ ˆ ˆ α2 = |l1 | d1 + d2 − µ − |l1 | α1 ; % (2, 2)-entry for i = 1 : n − 2 ˆi /αi ; mi = m ˆ id % (i + 2, i)-entry ∗ ∗ ˆ ˆ ˆ ˆ li+1 = (m ˆ i li di + li+1 di+1 − mi li αi )/αi+1 ; % (i + 2, i + 1)-entry ˆi + |ˆ ˆi+1 + d ˆi+2 − µ − |mi |2 αi − |li+1 |2 αi+1 ; αi+2 = |m ˆ i |2 d li+1 |2 d % (i + 2, i + 2)-entry end The computational cost of LDLH decomposition is 31n − 53 floating pointing multiplications and 17n − 28 floating pointing additions, a total of 48n − 81 floating operations (multiplications or additions). Similarly, we can compute the UDUH decomposition of P − µI as follows. Algorithm 2.2 Given the LDLH decomposition P = LDLH of a Hermitian pentadiagonal P , it computes the UDUH decomposition of the shifted P − µI = U DU U H . Let βn+2 = βn+1 = vn = vn−1 = un = 0. 3
A Twisted Factorization Method for Symmetric SVD of a Complex Symmetric Tridiagonal Matrix
1,2
Wei Xu1 and Sanzheng Qiao2 Department of Computing and Software, McMaster University Hamilton, Ont. L8S 4K1, Canada. 1 xuw5@mcmaster.ca 2 qiao@mcmaster.ca
1
using two-side Householder transformations or Lanczos method [6, 4]. In the second stage, the Takagi factorization T = V ΣV T of the complex symmetric tridiagonal T resulted from the first stage is computed. The methods for the second stage include the implicit QR method [1, 5] and the divide-andconquer method [7]. The QR method [1, 6] requires O(n2 ) flops for computing all singular values, but additional O(n3 ) flops for all the Takagi vectors. The divide-and-conquer method [7] integrates the computation of the singular values and the computation of the Takagi vectors using O(n3 ) flops. Thus, the main cost of the second stage is the computation of the Takagi vector matrix V . This paper presents a method for computing V given T and its computed singular values in Σ using O(n2 ) flops and n2 + O(n) storage space. In Section 2, we describe the twisted factorization, based on which, an efficient and stable method for computing the Takagi vectors is presented in Section 3. The accuracy and orthogonality of the computed vectors are analyzed in Section 4. Then, in Section 5, we deal with the case of multiple singular values. Our numerical experiment results in Section 6 show that our method is accurate and efficient.
