11.6. Fabrication of the Thermal Shields

合集下载

纺织材料学第一章英文

纺织材料学第一章英文To analyze and predict a fabric's performance, start with the fiber. Knowledge of fiber properties will help you understand the fiber's contribution to the performance of a fabric and the product made from it. Fiber properties are determined by their physical structure, chemical composition, and molecular arrangement.Some attributes of fibers are desirable and some are not. Thefollowing list of characteristics of a low-absorbency fiber includes consumer advantages and disadvantages:• Static cling• Rapid drying• Cool and slick hand• Poor skin-comfort—clammy• Waterborne soils do not stain• Evaporation of perspiration may occur by wicking, but fabric islow in absorbencyWick: 毛细管作用， wicking: 动名词。

• Dimensionally stable to water• G ood wrinkle recovery when laundered• Difficult to dye, but dyes are colorfast when launderedFast: 牢固的，耐久的， colorfast: 不褪色的。

专业英语

questions
How
do you distinguish steel from cast iron? How do you distinguish low alloy steel from high alloy steel?
1.1.1 Iron and Steel
The earth contains a large number of metals which are useful to man. One of the most important of these is iron. Modern industry needs considerable quantities of this metal, either in the form of iron or in the form of steel.
Mechanical Engineering materials
Organic polymer materials Inorganic non-metallic materials
plastic rubber Synthetic Fibers Traditional ceramics Special Ceramics Metal Matrix Composites
1.1.1 Iron and Steel
The ore becomes molten, and its oxides combine with carbon from the coke. The non-metallic constituents of the ore combine with the limestone to form a liquid slag. This floats on top of the molten iron, and passed out of the furnace through a tap. The metal which remains is pig iron.

Thermal Science and Engineering

Thermal Science and Engineering Thermal science and engineering is a captivating field that delves into the intricate world of heat transfer and its applications. It explores the fundamental principles governing the movement of thermal energy and seeks to harness this knowledge for the betterment of society. From the design of efficient power plants to the development of innovative cooling systems, thermal science plays a pivotal role in shaping our modern world. At the heart of thermal science lies the concept of heat transfer, the process by which thermal energy migrates from regions of higher temperature to those of lower temperature. This transfer can occur through three primary mechanisms: conduction, convection, and radiation. Conduction involves the transfer of heat through a material medium, such as a metal rod, while convection relies on the movement of fluids, like air or water, to carry heat away. Radiation, on the other hand, transmits heat in the form of electromagnetic waves, requiring no physical medium for propagation. The study of thermal science encompasses a wide range of disciplines, including thermodynamics, fluid mechanics, and heat transfer. Thermodynamics provides a framework for understanding the relationship between heat, work, temperature, and energy. Fluid mechanics deals with the behavior of fluids at rest and in motion, crucial for analyzing heat transfer in systems involving fluids. Heat transfer, as mentioned earlier, focuses on the mechanisms and rates of heat transfer between different objects or systems. Thermal engineering leverages the principles of thermal science to design, analyze, and optimize systems involving heat transfer. It encompasses a vast array of applications, including power generation, refrigeration, air conditioning, and materials processing. Power plants, for instance, rely on the principles of thermodynamics and heat transfer to convert thermal energy into electricity. Refrigeration and air conditioning systemsexploit the properties of refrigerants to transfer heat from one location to another, providing us with comfortable living and working environments. The advancements in thermal science and engineering have had a profound impact on our daily lives. They have enabled the development of more efficient and environmentally friendly power plants, reducing our reliance on fossil fuels. Innovations in refrigeration and air conditioning have improved food preservation,enhanced comfort levels, and facilitated the growth of various industries. Moreover, advances in materials processing, driven by thermal science, have led to the creation of new materials with exceptional properties, paving the way for technological breakthroughs. In conclusion, thermal science and engineering is an indispensable field that underpins numerous technological advancements andsocietal benefits. Its principles govern the movement of thermal energy, enabling us to harness this energy for various purposes. From power generation to refrigeration, from materials processing to environmental sustainability, thermal science plays a crucial role in shaping our world and improving our quality of life. As we continue to push the boundaries of knowledge and innovation, the field of thermal science and engineering holds immense promise for addressing future challenges and creating a more sustainable future.。

【优质】材料科学与工程专业英语第二版127101316课后习题翻译答案

【优质】材料科学与工程专业英语第二版127101316课后习题翻译答案Unit1：2.英译汉材料科学石器时代肉眼青铜器时代光学性质集成电路机械(力学)强度热导率1.材料科学指的是研究存于材料的结构和性能的相互关系。

相反，材料工程指的是，在基于材料结构和性能的相互关系的基础上，开发和设计预先设定好具备若干性能的材料。

2. 实际上，固体材料的所有重要性质可以概括分为六类：机械、电学、热学、磁学、光学和腐蚀降解性。

3. 除了结构和性质，材料科学和工程还有其他两个重要的组成部分：即加工和性能。

4. 工程师与科学家越熟悉材料的结构-性质之间的各种相互关系以及材料的加工技术，根据这些原则，他或她对材料的明智选择将越来越熟练和精确。

5. 只有在极少数情况下材料在具有最优或理想的综合性质。

因此，有必要对材料的性质进行平衡。

3. 汉译英Interdispline dielectric constantSolid materials heat capacityMechanical properties electro-magnetic radiationMaterials processing elasticity modulus1.直到最近，科学家才终于了解材料的结构要素与其特性之间的关系。

It was not until relatively recent times that scientists came to understand the relationship between the structural elements ofmaterials and their properties .2.材料工程学主要解决材料的制造问题和材料的应用问题。

Material engineering mainly solve the problems of materials processing and materials application.3.材料的加工过程不但决定了材料的结构，同时决定了材料的特征和性能。

材料科学与工程专业英语Unit2ClassificationofMaterials译文

Unit 2 Classification of MaterialsSolid materials have been conveniently grouped into three basic classifications: metals, ceramics, and polymers. This scheme is based primarily on chemical makeup and atomic structure, and most materials fall into one distinct grouping or another, although there are some intermediates. In addition, there are three other groups of important engineering materials —composites, semiconductors, and biomaterials.译文：译文：固体材料被便利的分为三个基本的类型：金属，陶瓷和聚合物。

固体材料被便利的分为三个基本的类型：金属，陶瓷和聚合物。

这个分类是首先基于这个分类是首先基于化学组成和原子结构来分的，化学组成和原子结构来分的，大多数材料落在明显的一个类别里面，大多数材料落在明显的一个类别里面，大多数材料落在明显的一个类别里面，尽管有许多中间品。

尽管有许多中间品。

除此之外，此之外，有三类其他重要的工程材料－复合材料，半导体材料和生物材料。

有三类其他重要的工程材料－复合材料，半导体材料和生物材料。

Composites consist of combinations of two or more different materials, whereas semiconductors are utilized because of their unusual electrical characteristics; biomaterials are implanted into the human body. A brief explanation of the material types and representative characteristics is offered next.译文：复合材料由两种或者两种以上不同的材料组成，然而半导体由于它们非同寻常的电学性质而得到使用；生物材料被移植进入人类的身体中。

Mathematical Modeling of Heat Transfer

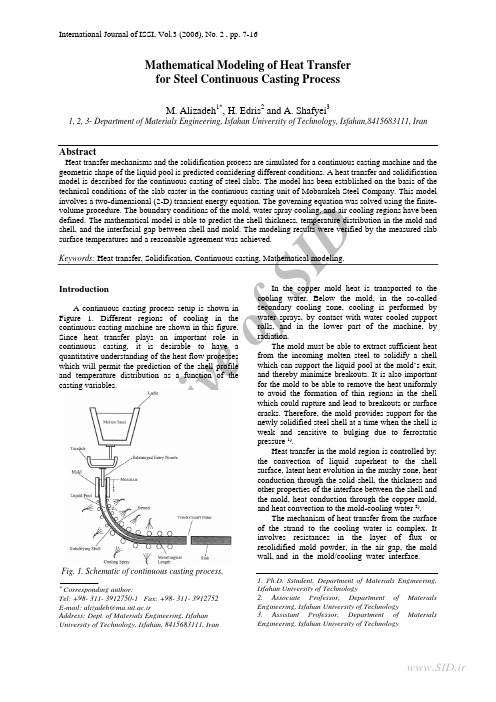
A rc hi v eo fSI DMathematical Modeling of Heat Transfer for Steel Continuous Casting ProcessM. Alizadeh 1*, H. Edris 2 and A. Shafyei 31, 2, 3- Department of Materials Engineering, Isfahan University of Technology, Isfahan,8415683111, IranAbstractHeat transfer mechanisms and the solidification process are simulated for a continuous casting machine and the geometric shape of the liquid pool is predicted considering different conditions. A heat transfer and solidification model is described for the continuous casting of steel slabs. The model has been established on the basis of the technical conditions of the slab caster in the continuous casting unit of Mobarakeh Steel Company. This model involves a two-dimensional (2-D) transient energy equation. The governing equation was solved using the finite-volume procedure. The boundary conditions of the mold, water spray cooling, and air cooling regions have been defined. The mathematical model is able to predict the shell thickness, temperature distribution in the mold and shell, and the interfacial gap between shell and mold. The modeling results were verified by the measured slab surface temperatures and a reasonable agreement was achieved.Keywords: Heat transfer, Solidification, Continuous casting, Mathematical modeling.IntroductionA continuous casting process setup is shown inFigure 1. Different regions of cooling in the continuous casting machine are shown in this figure.Since heat transfer plays an important role incontinuous casting, it is desirable to have aquantitative understanding of the heat flow processeswhich will permit the prediction of the shell profileand temperature distribution as a function of thecasting variables.Fig. 1. Schematic of continuous casting process.∗Corresponding author:Tel: +98- 311- 3912750-1 Fax: +98- 311- 3912752 E-mail: alizadeh@ma.iut.ac.irAddress: Dept. of Materials Engineering, Isfahan University of Technology, Isfahan, 8415683111, IranIn the copper mold heat is transported to the cooling water. Below the mold, in the so-called secondary cooling zone, cooling is performed by water sprays, by contact with water cooled supportrolls, and in the lower part of the machine, by radiation. The mold must be able to extract sufficient heat from the incoming molten steel to solidify a shell which can support the liquid pool at the mold’s exit, and thereby minimize breakouts. It is also important for the mold to be able to remove the heat uniformly to avoid the formation of thin regions in the shell which could rupture and lead to breakouts or surface cracks. Therefore, the mold provides support for the newly solidified steel shell at a time when the shell is weak and sensitive to bulging due to ferrostatic pressure 1).Heat transfer in the mold region is controlled by: the convection of liquid superheat to the shell surface, latent heat evolution in the mushy zone, heat conduction through the solid shell, the thickness and other properties of the interface between the shell and the mold, heat conduction through the copper mold, and heat convection to the mold-cooling water 2).The mechanism of heat transfer from the surface of the strand to the cooling water is complex. It involves resistances in the layer of flux or resolidified mold powder, in the air gap, the mold wall, and in the mold/cooling water interface.1. Ph.D. Sstudent, Department of Materials Engineering, Isfahan University of Technology2. Associate Professor, Department of Materials Engineering, Isfahan University of Technology3. Assistant Professor, Department of Materials Engineering, Isfahan University of TechnologyA rc hi v eo fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2Table 1: Comparison of thermal conductivities of materials present in the continuous casting mold [3].MaterialsTemperature(o C) Thermal conductivity(W m -1 K -1)Steel St 37 1200 29 Copper 30-130 385 Casting flux 1000-1300 0.5 to 1.2 Water 25 0.62Radiation conductivity of gas gap 1000 0.043Fig. 2. Different regions and simulation domain in continuous casting process.The thermal conductivities of different layers are compared in Table 1. As shown in this table, the air gap has the largest resistance to heat flow, while the other parts have a comparatively small resistance. Therefore, the pattern of heat removal in the mold is dependent largely upon the dynamics of gap formation. The air gap or contact resistances can be generated by the shrinkage of the steel shell away from the mold walls, especially after the flux is completely solid and unable to flow into the gaps. More researches were performed to show that the air gap was usually created in the lowest one-third of the mold length 3).In the upper part of the secondary cooling zone, the strand is usually sprayed by water emerging from nozzles arranged in the spaces between the rolls. The rate at which heat is extracted from the strand surface by water sprays has been measured by many researchers. These researchers have shown that under normal continuous casting conditions, in which shell surface temperatures range between 700 and 1200 o C, surface temperature has a little effect on the heat transfer coefficient. All studies agree that, in the stated temperature range, the spray water flux has the most effect on the heat transfer coefficient. Moreover, the temperature of sprayed water does not have a large influence on the heat transfer coefficient 4).In the lower part of the secondary cooling zone, heat transfer is preferred mainly by radiation and by roll contact. Therefore, it should be considered that the oxide scales generated on the surface of the strand could cause a thermal resistance in this zone 5).In this study, the mathematical model has been established on the basis of the technical conditions of the slab caster in the continuous casting unit of Mobarakeh Steel Company. In this model, steel heat capacity and steel thermal conductivity were considered as functions of steel temperature and chemical composition. Considering these functions, the governing equation is a non-linear equation. In this study the equation is solved in non-linear state. This model is also capable of predicting the temperature distribution, including the solidus and liquidus isotherm which defines the solid shell and mushy zone, respectively, as a function of section size, pouring temperature, steel composition, casting speed, mold length and spray conditions.Mathematical modelingFigure 2 shows the different regions of the continuous casting machine and the model considered for physical simulation of the caster. A typical method of modeling the strand thermal condition is shown in this figure. The mathematical model is applied to slices of strand that start at the meniscus and travel through the machine at the casting speed. New slices are generated periodically. A sufficient number of slices exist in each cooling zone to give an accurate representation of the thermal condition in each zone. In this model, only a quarter of the strand is considered due to the symmetry of the heat flow conditions (Figure 3).A rc hi v eo fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2Fig. 3. A symmetric quarter of strand width section with physical coordinate.AssumptionsThe following assumptions are made to simplify the mathematical model 6):-Conduction can take place only in the transverse directions.-Forced convective heat flow in the liquid pool is considered by defining an effective liquid thermal conductivity as:l eff K K ⋅=7(1) -The density of steel is constant, but specific heatcapacity and heat conductivity of steel are functions of temperature and chemical composition and therefore not constant.Model formulationThe energy conservation equation can be written as 7):(2)()()()()⎟⎠⎞⎜⎝⎛∂∂∂∂+⎟⎟⎠⎞⎜⎜⎝⎛∂∂∂∂+⎟⎠⎞⎜⎝⎛∂∂∂∂=∂∂+∂∂+∂∂+∂∂z T k z y T k y x T k x wH vH y uH x H t effeffeffρρρρ To simplify the equation, a transformation as wt z −=ζ is used. Therefore this is: (3)()ls ls s sH H H t y x H H q tHy H y x H x tH +==+∂∂−⎟⎟⎠⎞⎜⎜⎝⎛∂∂∂∂+⎟⎠⎞⎜⎝⎛∂∂∂∂=∂∂;,,,;0ζρααIn order to solve the governing equation, it is necessary to transform the physical domain into a computational domain. In general, this sort of transformation is used, and leads to a uniformly spaced grid in the computational domain but the points in physical domain may be unequally spaced. The original partial differential equation is transformed from physical coordinates (x, y ) to computational coordinates (ξ,η) by applying the chain rule of partial derivatives.;JS H H J H t x y s y x s s +⎟⎟⎠⎞⎜⎜⎝⎛∂∂∂∂+⎟⎟⎠⎞⎜⎜⎝⎛∂∂∂∂=⎟⎠⎞⎜⎝⎛∂∂ξηηαηηξξαξ (4);tH S l ∂∂−= y x J ηξ= In the above equations "S " is a term for heat source due to the metal phase transformation (liquid to solid). In order to establish the region of phase change, the latent heat contribution is specified as a function of temperature i.e. 8):f l l L f H ⋅=(5) Where L f is the latent heat of the phase changeand the liquid fraction (f l ) is computed by:⎪⎪⎭⎪⎪⎬⎫⎪⎪⎩⎪⎪⎨⎧≤≥≥−−≥=.......01sol sol liq sol liq sol liq l T T when T T T when T T T T T T when f (6)A typical 2D cartesian control volume is shownin Figure 4. This C.V contains a central node (P) with four neighborhood points (E, W, N, and S). The integral form of equation (4) is obtained on the control volume by the finite volume method 9,10): a P H sP = a E H sE + a W H sW + a N H sN + a S H sS + b (7) a P = a W + a E + a S + a N + a P oa E =ey x e ⎟⎟⎠⎞⎜⎜⎝⎛ΔΔηξξηα a W =wyx w ⎟⎟⎠⎞⎜⎜⎝⎛ΔΔηξξηα a N =nx y n ⎟⎟⎠⎞⎜⎜⎝⎛ΔΔξηηξα a S =sx ys ⎟⎟⎠⎞⎜⎜⎝⎛ΔΔξηηξαa P o =PJ t ⋅ΔΔ⋅Δηξ b = a P o (H sP o + H lP o - H lP ) + o Pq J ρηξΔ⋅Δ (8)Fig. 4. A typical control volume and the notation used for a Cartesian 2D grid [9].To approximate the variable values on the surface of the control volume, the Quadratic Upwind Interpolation (QUICK) algorithm is used 9). In the QUICK scheme, the variable profile between two points approximated by a parabola instead of aArc hi v eo fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2straight line (Figure 5), on a uniform Cartesian grid leads to 9):W E P e φφφφ818386−+= (9)Fig. 5. Approximation of gradients at cell faces [9].Boundary conditionsTo solve the above equation, the boundary conditions are needed for different regions include the mold, water spray cooling, and air cooling. Figure 6 shows some machine cooling layouts while the technical information belonging to each zone is shown in Table 2. A general form of the boundary condition can be expressed through an equation (10), in which the heat transfer coefficient, h , is estimated for different cooling zones.()water b b T T h n T k −=∂∂−| (10)To determine the temperature of the boundaries, the discretion of the bnT |∂∂ on boundary points isrequired. According to the QUICK scheme b nT |∂∂could be calculated by the following correlation [10]: nT T T nT W P b bΔ+−=∂∂398(11)Therefore, as seen in equation (10), finding the heat transfer coefficient for different regions such as: mold, water spray, and air cooling is necessary.Fig. 6. Secondary cooling of the slab caster.In the mold, several thermal resistance layers exist between the steel shell surface and the recirculation water. All the thermal resistances in the mold are shown in Figure 7. The effective thermal resistance of the water channel is estimated from the water channel heat transfer coefficient, thermal conductivity and the thickness of scale deposits on the surface of the cooling-water channel 11):⎟⎟⎠⎞⎜⎜⎝⎛+=w scale scale water h k d r 1 (12)Fig. 7. Thermal resistances existing between the shell surface and water channel in the mold.Table 2. Secondary cooling zones variables.No. Zone Length zone(m)SegmentNumber of spray nozzles Water flow rate(m 3/sec)Number rollin zoneRoll radius (m)1 0.439 - -2 0.220 - -3 0.303 - 30 0.003975 - -4 0.925 0 38 0.004967 5 0.140 5 1.470 0 38 0.0048426 0.200 6 1.475 1 10 0.004858 5 0.2507 1.725 2 10 0.003975 5 0.3008 1.725 3 10 0.003733 5 0.3009 3.950 4,5 20 0.005667 10 0.350 10 5.200 Roll 37-47 22 0.006483 11 0.380,0.440 11 9.400 Roll 48-63 - Air cooling 16 0.440A rc hi v eo fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2 The heat transfer coefficient between the water and the side walls of the water channel (h w ) is calculated assuming a turbulent flow through an equivalent-diameter pipe (D ) using the empirical correlation of Sleicher and Reusse 11):()21Pr Re 015.05c c water w Dkh += (13)()Pr 6.0215.0333.0;Pr 424.088.0−+=+−=e c cOther thermal resistances shown in Fig 7 can be calculated by the following correlations [11]:;;slag slag slag moldmold mold k d r k dr == ;air air air k d r = (14)Which d slag can be found from the powder consumption per mass of product (M slag (kg/ton)): ()N W N W M d slagsteel slag slag +×××=2ρρ (15) Also, d air includes a gap due to shrinkage of the steel shell, which can be calculated by the thermal linear expansion relation of steel using following correlation for each control volume: T l l Δ⋅⋅=Δλ (16) Where, λ is linear thermal expansion coefficient of steel and l is length of each control volume. Moreover, the heat transfer coefficient due to radiation is calculated by: ()()212221T T T T h rad ++=εσ (17) Heat transfer mechanisms in the spray cooling zones below the mold are defined in Figure 8. The heat extraction due to the water sprays is a function of the water flux. The relationship between the rate of heat extraction by the water sprays and the spray variables has been established in a number ofexperimental studies. One of the most widely usedrelations has been presented by Nozaki's 12): ();0075.013925.055.0water water spray T Q h ×−××= (18)Fig. 8. Heat transfer mechanisms in the secondarycooling zones [12].Due to the high temperature of the strand surfaceand the exposure of water to the surface, an oxidescale is produced on the surface of the strand. Despite the low thickness, the scale can have animportant role in the heat transfer control. Therefore,the effective heat transfer coefficient should be considered as 12):sprayscsceff h k h 11+=δ (19)It has already been mentioned that the cooling ofthe strand in the lower part of the secondary cooling zone is mainly done by radiation 12). Therefore, the equation for the heat transfer coefficient is given as follows:()().2.2am s am s rad T T T T h ++=εσ (20) Besides the radiation, heat transfer is alsoachieved by natural convections, but this part is rather small and can be neglected in comparison to radiation cooling.The symmetrical boundary condition has been considered for midplanes as follows:0.,00.,0=∂∂−==∂∂−=yTk y xTk x (21)Computation and verificationThe algebraic equation of the boundary conditions has been solved with a Tridiagonal Matrix Algorithm (TDMA) solver 13). As seen in equations(7) and (8), to solve the algebraic equation, it is necessary to know the latent enthalpy in a new time step (H l ). To update the amount of the latent enthalpy an iterative solution is used for each time step:11++−+=k sP k sP k lP k lP H H H H (22)As seen in the above equation, by using the sensitive enthalpy that has been obtained by solving the energy equation (H sP k+1), latent enthalpy could be updated in the k+1th inner iteration in each time stepto achieve a certain convergence. The calculation mentioned has been programmed in the FORTRAN language. The mathematic simulation starts by setting the initial steel temperature at the pouring temperature. Input parameters, in Table 3, in the standard cases are verified by the measured temperatures on the shell surface of the strand.Equilibrium lever-rule calculations are performed on a Fe-C phase diagram in order to calculate steel phase fractions. By this means, phase field lines are specified as simple linear functions of carbon equivalent content. The carbon of the steel is applied as the carbon equivalent content that is calculated by the following correlation 14): wt%CE=wt%C+0.04(wt%Mn)-0.14(wt%Si)-0.04(wt%Cr)-0.1(wt%Mo)-0.24(wt%Ti)+0.1(wt%Cu) For a 0.16%C, 1.3%Mn, 0.5%Si, 0.05%Cr, 0.03%Mo and 0.01%Ti plain carbon steel, the carbon equivalent percentage calculated as 0.135 and also the equilibrium phase diagram model calculates T liq.=1528 o C, T sol = 1494 o C. The solid fraction-A rc hi v eo fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2temperature curve in the mushy zone obtained from the model is shown in Figure 9. As seen in this figure the relation between temperature and solid fraction of steel in the mushy zone is non-linear.Solidified shell thickness is one of the most important calculated parameters in the model, and the influence of the grid spacing on this parameter should be considered. The influence of the gridspacing on the solidified shell thickness, in exit point of the mold, is shown in Table 4. The thickness amounts in width and narrow sides are presented in this table. It is clear from Table 4 that when grid spacing is reduced, solidified thicknesses are changed and they are stable in a narrow limit with reduction of grid spacing lower than a certain limit. It can be concluded that the solidified shell thickness is independent of the mesh size with the reduction of grid spacing lower than a certain limit.The mold zone is a complex and important area in continuous casting machine. The solid shell growth in this zone is complicated and the results of this study are compared to those of some other researchers. The comparison between the results of the model in this study and experimentalmeasurement by some other researchers is shown in Figure 10. The figure shows the variation of growth of the solid shell thickness on the ingot for low carbon steel (0.06% C). It is clear that the results from numerical solution in this study have a very good compatibility with those of three 3-D model of Thomas and experimental measurement of Alberny and co-worker.Table 3. Input data for standard conditions.Carbon equivalent content, CE pct 0.132 pctSteel density, ρ7500 kg/m 3 Steel emissivity, ε0.8 Mold copper plates thickness 0.043×0.030 m ×m Total mold length 0.704 m Mold copper plates width 2.220×0.215 m ×m Scale thickness on the surface of mold cold face 0.00001 m Mold conductivity, k mold 315 W/mK Mold powder conductivity, k slag 1.27 W/mK Air conductivity, k air 0.083 W/mKMold powder density, ρslag0.650 kg/m 3 Mold powder consumption rate, M slag 0.8 kg/ton steel Casting speed, V c 0.0167 m/secPour temperature, T in 1546 oCLiquidus temperature, T liq. 1528.6 oCSolidus temperature, T sol. 1494 oC Working mold length 0.659 m Slab geometry, W ×N 1.250×0.203 m ×m Scale conductivity on the surface of slab, k sc 0.5 W/mK Scale conductivity on the surface of mold, k scale 1.0 W/mK Water channel geometry, large & small plates 25×5×29 & 22×5×26mm 3 Average cooling water temperature in the mold 28 oC Water flow rate entering the mold small plate, 0.0061 m 3/sec Water flow rate entering the mold large plate, 0.0553 m 3/sec Latent heat of the steel phase change, L f 272140 J/kgWater conductivity , k water0.615 W/mKSteel specific heat capacity [11], C p ()()()()⎪⎪⎪⎪⎪⎪⎭⎪⎪⎪⎪⎪⎪⎬⎫⎪⎪⎪⎪⎪⎪⎩⎪⎪⎪⎪⎪⎪⎨⎧≥=≤=≤+=≤=−==+=+=liqp liq sol p sol op p o p p op o p T T C T T T C T T C T C T C T C T C T C T C T C T C T C 7877721100*334.02681100850648850750*766.338497507001431700500*836.0268500*376.0456p p p p p p p p p p J/kgK Steel conductivity, k steelf l *k liq +(1-f l )*k sol W/mK Solid steel conductivity, k sol 33.0 W/mK Effective molten steel conductivity, k liq 7*43.0 W/mKScale thickness on the surface of slab , δsc0.001 mA rc hi v eo fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2Table 4. Effect of mesh size on the shell thickness at mold exit.Grid system #nx ×n y Shell thickness at middle of wide face (m)Shell thickness at middle of narrow face (m)25×10 0.0145 0.0111 25×15 0.0135 0.0110 50×15 0.0136 0.0118 50×20 0.0133 0.0119 100×200.0136 0.0117 100×300.0131 0.0117Fig. 9. Phase fraction variation with temperature in mushy zone.Fig. 10. Comparison between model results and other references.Results and discussionThe calculated surface temperatures of a slab, for the Table 3 conditions as a function of the distance below the meniscus, are presented in Figure 11. This figure shows the calculated surface temperatures at the centers of the wide and narrow faces and at the corners of the slab caster. The central areas of the wide faces are cooled one-dimensionally, whereas the slab corners are subject to 2-D cooling. The slab corners can therefore become significantly colder than other parts of the inner wide face. At the beginning of straightening, the slab corner temperature is 230 o C less than the temperature at the center of the wide face. Control of corner cooling is critical for much of continuously cast products. The slab corners tend to have meniscus marks, which act as stress risers. A combination of temperature, stress risers and a low ductility region in the 700-900 o C temperature range during the straightening process often leads to cracks in the corners. One way to increase the corner temperature is to widen the strip of unsprayed strand at the corner. However, as the non-sprayed strip is widened, a hot spot will develop between the colder corner and the sprayed area. Thus, the design must be in a way to ensure that it does not cause other quality problems. Also, as seen in Figure 11, the intensity of heat transfer of mist spray cooling is less than cooling in the lower part of the mold for all of the curves, because of this, the model predicts a 200 o C reheating of the slab surfaceon leaving the mold. A similar situation also exists in water spray cooling and air cooling regions on the surface of the wide face slab.Fig. 11. Predicted surface temperature of strand. Figure 12 shows the solidified shell thickness profiles of both the narrow and wide faces of the slab. This figure shows a sudden change of slope at the beginning of the solidified shell growth curve. It is indicated that the rate of solidified shell growth is clearly high in the mold region. Figure 13 shows a set of data of the local heat flux density along the length of the mold [15]. There is a maximum of heat flux density somewhat below the meniscus. Downward, the heat flux density usually decreases. If the air gapA rc hi v eIDInternational Journal of ISSI, Vol.3 (2006), No. 2between the mold and strand approximately has uniform thickness, or it increases uniformly in the downward direction, the heat flux density along the length of the mold continuously decreases.Fig. 12. Predicted solidified shell thickness.Fig. 13. Heat flux density distribution along the length of the mold.Fig. 14. Effect of casting speed on the pool depth.The casting speed is the most effective parameter in changing the position of the solidified shell thickness profiles. The relation of the "metallurgical length" (maximum length of the liquid pool) with the casting speed is shown in Figure 14. Increase in casting speed decreases the holding time of the slab in the secondary cooling zones and increases the length of the liquid core. Therefore, casting speed is the most important factor in controlling mold heat extraction. The solidified shell thickness as a function of the casting speed of bothwide face and narrow face of the strand are shown in Figure 15. Since, a lower casting speed provides more time for the heat to be extracted from the shell, the shell thickness increases. Moreover, the shell thickness in the initial solidification stage decreases at the high casting speed, as shown in Figure 15, which often easily causes the breakout of strand. Therefore, to prevent this unfavorable defect, the casting speed is limited. It should be mentioned that steel composition and slab width in comparison to casting speed has no significant effect on the shell thickness. As seen in this figure, solidified shell thickness for wide face is higher than it is for narrow face. This is because of the different air gap sizes between mold powder and mold wall in both wide and narrow faces. The air gap size along the length of the mold is predicted by the model for both faces in Figure 16. As seen in this figure, total shrinkage value in narrow face is more than wide face as expected because width size of slab is much larger than thickness size in cross section of strand. Furthermore, this phenomenon could change Fig. 15. Effect of casting speed on shell thickness.Fig. 16. air gap size along the mold.Heat transfer in the mold is governed by these three resistances: the casting-mold interface, the mold wall, and the mold-cooling water interface. Although, thermal resistance due to the air gapA rc hi v e o fSI DInternational Journal of ISSI, Vol.3 (2006), No. 2 should also be considered while the amount of air gap thermal resistance is usually quite large compared to the other resistances especially for the lower portion of the mold. Temperatures of three points: slag layer/mold wall interface (T 1), mold wall (T 2), and water channel wall (T 3) as shown in Figure 7 are predicted by the model. Since, the heat flux for steady state conditions will be constant and independent of distance: (23)mold rad airradair slag s rad airrad air slags slags total water s r rr r r r T T rr r r r T T r T T r T T q +⎟⎟⎠⎞⎜⎜⎝⎛+⋅+−=⎟⎟⎠⎞⎜⎜⎝⎛+⋅+−=−=−=′321The above relation represents three equations for the three unknowns, T 1, T 2, and T 3. The results of the model predictions are shown in Figure 17(a) and Figure 17(b). As seen in these figures, the existence of the air gap between the shell surface and mold wall causes the T 1 to increase both in wide and narrow faces of strand. Since the air gap thickness in the narrow face is larger than the wide face, T 1 in the narrow face is much higher than in the wide face. Therefore, the pattern of heat removal in the mold is dependent largely upon the dynamics of gap formation. It causes temperature difference to exist in solidified shell and has a strong influence on transverse cracks generation in the mold region.Fig. 17. Predicted temperatures for T 1, T 2 and T 3 points in Fig 7 -(a) wide face and (b) narrow face (T 1=slag layer/mold wall interface temperature, T 2=mold wall temperature, and T 3=water channel wall temperature).The effect of the amount of superheat temperature of the molten steel, entering the mold, on the solidified shell thickness is shown in Figure 18. At first, with increasing the superheat temperature, the superheat flux will increase and therefore, the solidification rate decreases. This, in turn, reduces the thickness of the steel shell. On the other hand, by increasing the superheat temperature, the driving force for the heat transfer will increase. Therefore, as seen in Figure 18, the influence of the superheat temperature is insignificant to shell growth, especially in the wide face of the strand. Low superheat, near liquidus temperature, is beneficial to continuous casting. Internal quality is improved as the equated zone is made significantly larger. Therefore, a more desirable structure with greater resistance to halfway cracks is produced. Centerline segregation and porosity are also reduced or eliminated.Fig. 18. Effect of steel superheat temperature on the shell thickness at mold exit.Conclusions1- A finite volume heat transfer and solidification model has been formulated to predict the temperature field and liquid pool position in the continuous casting process under different conditions. This has been verified with the temperature measurement of slab surface.2- Casting speed is the most effective parameter on mold heat removal. Therefore, it is the most important factor in controlling solidified shell thickness and slab temperature.3- Since, the air gap size in narrow face of mold is higher than the wide face, the breakout of strand often occurs in the narrow face.4- Air gap existing in the casting-mold interface causes a large thermal resistance for heat transfer from the solidified shell to the mold. Therefore, it has a strong influence on product quality and casting problems, especially for the narrow face of strand. 5- High superheat temperature may cause breakouts at the mold exit, especially for the narrow face, so it should be exactly kept at a low level.。

The Chemistry of Construction Materials

The Chemistry of ConstructionMaterialsConstruction materials, like concrete, steel, and wood, are essential in building structures for residential, commercial, and industrial purposes. At their core, these materials rely on chemical reactions to form and maintain their structures.Concrete, for example, is made by mixing and reacting cement, water, and aggregates. Cement, the primary binder in concrete, is manufactured by heating a mixture of limestone and clay at high temperatures to form clinker, which is then ground into fine powder and mixed with gypsum to make cement. When these components are mixed with water, the cement undergoes a chemical reaction called hydration, producing a calcium silicate hydrate gel that hardens and binds the aggregates together to form solid concrete. The chemical composition and proportion of cement, water, and aggregates affects the strength, durability, and workability of the concrete.Steel, another widely used construction material, is an alloy of iron and carbon. The addition of other elements, such as manganese, chromium, and nickel, can enhance steel’s properties, such as strength, toughness, and corrosion resistance. Steel manufacturing involves several chemical reactions, including the reduction of iron ore with carbon in a blast furnace and the refining and further processing of the molten iron into steel. Chemical coatings, such as galvanizing and painting, can also be applied to steel to protect it from rust and other environmental factors.Wood, a renewable construction material, is composed of cellulose, hemicellulose, and lignin, which are polymers made of carbon, hydrogen, and oxygen. These polymers provide wood with its strength and flexibility. Wood’s properties, such as moisture content, density, and grain direction, affect its performance and durability. Wood can also be treated with chemical preservatives, such as copper-based compounds, to prevent decay and insect infestation.In addition to these traditional construction materials, there are also emerging materials that rely on chemical reactions for their properties. For example, self-healing concrete contains capsules filled with a healing agent that can repair cracks when they form. The agent reacts with the moisture in the concrete to form a solid precipitate that fills the crack and restores the concrete’s strength.In conclusion, the chemistry of construction materials plays a critical role in the design and performance of structures. By understanding the chemical reactions and properties of these materials, engineers and architects can select the appropriate materials and ensure the safety, durability, and sustainability of their designs. The future of construction materials will undoubtedly involve new innovations and advancements, and it will be exciting to see how chemical reactions will continue to shape this field.。

机械英语考试试题及答案

机械英语考试试题及答案一、选择题（每题2分，共20分）1. The term "mechanical engineering" refers to:A. The study of machinesB. The design and manufacture of mechanical systemsC. The operation of machineryD. The maintenance of mechanical equipment答案：B2. What is the function of a bearing in a mechanical system?A. To reduce frictionB. To increase efficiencyC. To provide powerD. To transmit motion答案：A3. The process of converting thermal energy into mechanical energy is known as:A. ElectrificationB. CombustionC. ThermodynamicsD. Hydrodynamics答案：C4. In mechanical design, the principle of "KISS" stands for:A. Keep It Simple, StupidB. Keep It Short and SimpleC. Keep It Simple and SafeD. Keep It Simple, Smart答案：A5. A gear train is used to:A. Change the direction of motionB. Increase the speed of rotationC. Decrease the speed of rotationD. All of the above答案：D6. What does CAD stand for in mechanical engineering?A. Computer-Aided DesignB. Computer-Aided DraftingC. Computer-Aided DevelopmentD. Computer-Aided Diagnostics答案：A7. The SI unit for force is:A. NewtonB. JouleC. PascalD. Watt答案：A8. What is the purpose of a flywheel in a mechanical system?A. To store energyB. To increase speedC. To reduce noiseD. To dissipate heat答案：A9. The term "hydraulics" is associated with the study of:A. Fluid dynamicsB. Solid mechanicsC. Structural analysisD. Thermal engineering答案：A10. The process of cutting a material to a specific shape is known as:A. MachiningB. CastingC. ForgingD. Extrusion答案：A二、填空题（每空1分，共10分）11. The formula for calculating the moment of a force is $ F \times d $, where $ F $ is the force and $ d $ is the_______.答案：distance from the pivot12. A _______ is a device that converts linear motion into rotational motion.答案：crank13. In a four-stroke internal combustion engine, the four strokes are intake, compression, _______, and exhaust.答案：power14. The _______ of a material is its ability to resist deformation under load.答案：stiffness15. The term "overhaul" in mechanical maintenance refers to a thorough inspection and _______ of a machine or its parts.答案：repair16. The _______ of a machine is the study of how forces act on and within a body.答案: statics17. A _______ is a type of machine that uses a screw to convert rotational motion into linear motion.答案：screw jack18. The _______ of a system is the point around which the system rotates.答案：pivot19. The _______ of a lever is the ratio of the effort arm to the load arm.答案：mechanical advantage20. The _______ is a type of bearing that allows for rotation with minimal friction.答案：ball bearing三、简答题（每题5分，共30分）21. Explain the difference between static and dynamic equilibrium in mechanical systems.答案：Static equilibrium refers to a state where the net force and net moment acting on a body are zero, resulting in no acceleration. Dynamic equilibrium occurs when the net force is zero, but the body is in motion with constant velocity.22. What is the purpose of a clutch in a vehicle?答案：A clutch is used to engage and disengage the power transmission from the engine to the transmission system, allowing the vehicle to start, stop, and change gears smoothly.23. Describe the function of a governor in an engine.答案：A governor is a device that automatically controls the speed of an engine by regulating the fuel supply or the valve settings, ensuring the engine operates within safespeed limits.24. What are the three primary types of joints in structural engineering?答案：The three primary types of joints are pinned joints, fixed joints, and sliding joints, each serving different purposes in connecting and supporting structural elements.25. Explain the。

混凝土热开裂数值描述方法1(英文)

Thermal induced stress and associated crackingin cement-based composite at elevated temperatures––Part I:Thermal cracking around single inclusionY.F.Fu a ,Y.L.Wonga,*,C.A.Tang b ,C.S.PoonaaDepartment of Civil and Structural Engineering,The Hong Kong Polytechnic University,Hong Kong,ChinabLab of Numerical Test of Material Failure,Northeastern University,Shenyang 110006,ChinaAccepted 25April 2003AbstractThis paper presents the development and veriﬁcation of 2-D mesoscopic thermoelastic damage model used to numerically quantify the thermal stresses and crack development of a cement-based composite subjected to elevated temperatures.The program is then used to study the thermal fracture behavior of a cement-based matrix with a single inclusion.The results show that the mechanisms of thermal damage and fracture of the composite depend on (i)the diﬀerence between the coeﬃcients of thermal ex-pansion (CTE)of the inclusion and the cement-based matrix,(ii)the strengths of materials,and (iii)the heterogeneity of materials at meso-scale.The thermal cracking is an evolution process from diﬀused damage,nucleation,and ﬁnally linkage of cracks.If the CTE of the inclusion is greater than that of the matrix,radial cracks will form in the matrix.On the other hand,inclusion cracks and tangential cracks at the interface between inclusion and matrix will form if the CTE of the inclusion is smaller than that of the matrix.Ó2003Elsevier Ltd.All rights reserved.Keywords:Thermal stress;Thermal induced cracking;Heterogeneity;Numerical simulation1.IntroductionThermal cracking induced by thermal mismatch has been one of the problems in a cement-based composite material under elevated temperatures.For a multi-phase material,the eigenstrains deriving from the heteroge-neous deformations among phase components inevita-bly cause cracking in the composite,even though it is under a uniform temperature ﬁeld.Experimental results [1]have shown that this type of cracking signiﬁcantly reduces the strength and elastic modulus of a cement-based composite.However,the entire thermal cracking process (initiation,propagation and linkage of cracks)and the associated stress distributions under elevated temperatures are diﬃcult to quantify experimentally,mainly because of the limitation of equipment and the complex structure of a composite material.In order to understand the failure mechanism of a composite material due to thermal eﬀects,many math-ematical models have been proposed [1–3].In these models,the driving stresses for the crack initiation and propagation are the heterogeneous eigenstresses,which develop in and around the restraining inclusion.These eigenstresses might be caused by thermal expansion,shrinkage [4,5],initial strains and misﬁt strains.Timo-shenko and Goodier in 1970[6]proposed a closed-form solution for the axisymmetric problem of a circular in-clusion concentrically embedded in the circular disc of another phase material with diﬀerent thermal and mechanical properties.Hsueh et al.[7]transformed a composite with a microstructure of square-array,hexagon-array,brick-array grains,as well as the actual microstructure of random-array grains into a simple composite-circle analytical model.The residual thermal stresses were predicted reasonably well using the pro-posed linear elastic solutions except for the model mi-crostructure of brick-array grains.A modiﬁed version of Timoshenko and Goodier Õs solution incorporating the longitudinal strain proposed by Gentry and Husain*Corresponding author.Tel.:+852-2766-6009;fax:+852-2334-6389.E-mail address:ceylwong@.hk (Y.L.Wong).0958-9465/$-see front matter Ó2003Elsevier Ltd.All rights reserved.doi:10.1016/S0958-9465(03)00086-6Cement &Concrete Composites 26(2004)99–111[2]was also used to study the diﬀerential pressure de-veloped in the interface between concrete and a com-posite rod.As for a40°C temperature increase,the concrete was modeled with a linear-elastic and nonlin-ear tension-softening material model using aﬁnite ele-ment approach.The calculated results showed that the large spacing of the rods and the thick concrete cover were helpful to reduce the tensile stress in concrete as well as the potential for thermally induced cracking. Based on a fracture mechanics model,Timoshenko and GoodierÕs solution was adopted by Dela and Stang[3] to calculate the crack growth with time in a high-shrinkage cement paste with a single aggregate disc.The experimentally measured stresses in the selected circular aggregate were employed to predict the stresses dis-tributed in cement paste and the crack growth at a crack tip close to the aggregate in terms of a given stress in-tensity factor.Although the above-mentioned models deepen the understanding on thermal stress and cracking,essen-tially,none of them can simulate the entire thermal cracking process from crack initiation to propagation. HsuehÕs and RussellÕs models can determine the stress distribution around a single inclusion in the composite before crack initiates.DelaÕs model was suitable to cal-culate the critical stress value when an existing crack starts to grow.The stress distribution represented by this model would be invalid as soon as the crack is ex-tended.A fracture mechanics model is able to study the growth of existing single crack,but it is not suitable to explain the initiation and coalescence of cracks.More importantly,the phase materials of a cement-based composite are often heterogeneous so that the eﬀect of change in microstructure(mesostructure)on the mac-roscopic behavior is diﬃcult to be studied by using an analytical model.Consequently,a numerical method appears to be an eﬀective tool to model cracking processes.Substantial progress[8,9]has been achieved in numerical simulation of failure occurring in a cement-based composite at ambient temperatures.However,a satisfactory model to simulate the cracking processes caused by the thermal induced stresses in a heated cement-based composite is still not available.The aim of this paper is to propose and verify a mesoscopic thermoelastic damage(MTED)model,that can numerically simulate the formation,extension and coalescence of cracks in a cement-based composite ma-terial(cement-based matrix+aggregate inclusion), caused by the thermal mismatch of the matrix and the inclusion under uniform temperature variations and free boundary conditions.Numerical studies of the eﬀects of the thermal mismatch between the matrix and a single circular inclusion on the stress distribution and crack development are also presented.2.Numerical modelIn the MTED model,phase materials of a composite are considered to be heterogeneous following the Wei-bull distribution.Tensile and shear cracking at meso-scale occur if the stress in the composite subjected to high temperatures satisﬁed with the failure criteria of Coulomb–Mohr with tensioncutoﬀ. 100Y.F.Fu et al./Cement&Concrete Composites26(2004)99–1112.1.Material modelFor a cement-based composite material,the phase materials are cement mortar matrix and aggregate in-clusions.Although the composite material is regarded as an isotropic elastic-brittle solid at a macroscopic scale, while the individual grains in the matrix and inclusions are distinguished at microscopic or mesoscopic scales [8].The eﬀect of heterogeneity on the stress distribution has been studied[10],and much of the behavior ob-served at a macro-level can be explained in terms of the material structure at a meso-level.As a result,the matrix and the inclusions are considered as disorder solids in a meso-scale in this study.To account for the heterogeneity of the matrix and inclusions,their statistical distributions of properties (elastic modulus,compressive strength and Poisson ratio)are assumed to follow the Weibull distribution:uðh;bÞ¼hb0Ább0hÀ1ÁeÀðb=b0Þhð1aÞwhere uðh;bÞis the distribution density of parameter b which is a material property(such as strength,elasticity and Poisson ratio)of a representative volume element (RVE)in the mesh divisions,and b0is the mean value of the material property under consideration.h is the ho-mogeneity index of the RVE which represents the degree of homogeneity.The statistical distribution function Uðh;bÞis expressed by Eq.(1b)after integrating Eq.(1a):Uðh;bÞ¼1ÀeÀðb=b0Þhð1bÞThe randomness of the mechanical properties of RVE can be simulated using the distribution function with given parameters h and b0,i.e.Uðh;b0Þ.The relationship of distribution density of RVE strength and homoge-neity index is shown in Fig.1.With increasing h,the material is more homogeneous or vice versa.For in-stance,we consider a material with a mean strength of 200MPa.If the material has a homogeneity indexðhÞof 30,the distribution probabilities Uð30;200Þwill be close to zero and unity for the strengths of RVE less than160 and210MPa,respectively as shown in Fig.1b.In an-other case,if it has a homogeneity indexðhÞof1.1,the corresponding distribution probabilities Uð1:1;200Þwill become0.54and0.64for the strengths of RVE less than 160and210MPa,respectively.From these strength distributions,it is evident that increasing heterogeneity of a material will increase both the diﬀerence in me-chanical properties among the RVEs,and the popula-tion of the RVE with lower strengths.The strength,elastic modulus and Poisson ratio are randomly allocated to each RVE so to account for the inherent variability in phase materials,using the Monte-Carlo method.A more detailed introduction and ex-planation to the material model were reported in our previous publications[11–13].The thermal properties (CTE)of the phase materials are assumed to be uniform and location-invariant,and only depend on the indi-vidual phase.2.2.Mesoscopic thermoelastic damage(MTED)modelIt has been known that the thermal damages of a heated concrete is a complex problem.There are a number of aﬀecting factors,such as thermal mismatch, temperature gradient,degradation of mechanical prop-erties of cementitious materials due to chemical de-composition,and pore water pressure,that cause such damages.However,the focus of this paper is on the damage caused by diﬀerential thermal strains as aresultof diﬀerent CTEs of the phase materials(matrix and inclusion).Studies[8,14]show that the macroscopic fracture of materials is always related to the initiation and propagation of cracks at a meso-scale.Hence,it is assumed that the damage of a cement-based composite is due to the cracking caused by thermal induced stresses at a meso-scale.The bond between the matrix and the inclusion is considered to be perfect.In fact,the pro-posed model can be further modiﬁed to incorporate the eﬀects of temperature gradients and temperature-de-pendent mechanical properties,pending on the avail-ability of experimental data to quantify the associated simulation functions,details of which are under inves-tigation by the authors of this paper.In the numerical modeling,each phase material is discretized into many RVEs with a suitable charac-teristic length.In general,the precision of computa-tional results will increase with decreasing RVE size,at the expense of longer computational time.The RVE has the same size as the meshedﬁnite element in this paper.It is also assumed that the stress–strain rela-tionship of a RVE is linearly elastic till its peak-strength is reached,and thereafter follows an abrupt drop to its residual strength.Cracking is treated as a smeared phenomenon.That is,a crack is not consid-ered as a discrete displacement jump,but rather changing the properties of the RVE according to a continuum law,such as damage mechanics.Although this modeling approach might appear to be crude, however,the complex failure phenomenon(such as compressive and tensile failure)and the nonlinear be-havior in a macro-scale have been proved to be suc-cessfully simulated using the material heterogeneity [11].The behavior laws of the RVE are implemented by introducing a MTED variable D into a constitutive relationship.Based on the above-mentioned ideas and the damage mechanics[21],the general form of an eﬀective stress for a given state of damage for a RVE can be expressed as follows:r¼ð1ÀDÞÁE0Áe rð2Þwhere r is the eﬀective stress,D is the damage variable, E0is the elastic modulus at a reference/undamaged condition(such as at reference temperature),and e r is the strain.Under a uniform temperatureﬁeld,the damage is induced both by diﬀerential thermal strains and by the temperature increment D T,the general ex-pression of damage variable is D¼Dðe r;D TÞ.Let D m and D T denote the damages by the thermal strain and temperature increment,respectively.They can be ex-pressed in terms of the stiﬀness degradation as follows: D m¼1ÀEðe rÞE0ð3ÞD T¼1ÀEðD TÞE0ð4Þwhere Eðe rÞand EðD TÞare the elastic modulus corre-sponding to a given thermal strain e r and the elastic modulus at temperature increment of D T,respectively. If they are independent,the damage variable Dðe r;D TÞcan be expressed as follow:Dðe r;D TÞ¼1Àð1ÀD mÞÁð1ÀD TÞDðe r;D TÞ¼1ÀEðe rÞE0ÁEðD TÞE0ð5ÞSince the temperature-dependent properties are not considered,the damage D T is equal to zero.According to the description of the damage process of a material by Mazars[14]and Yu[15],the thermal induced damage before and after the peak-strength can be determined by the thermal strain and the temperature increment D T102Y.F.Fu et al./Cement&Concrete Composites26(2004)99–111through a separation function,respectively.Fig.2shows a general constitutive relationship of a RVE under thermal loading.At a temperature increment of D T ,the initial thermal strain e thermal is equal to a ÁD T ,and the damage at any given thermal strain can be calculated from Eq.(6)D ðe ;D T Þ¼0;e thermal 6e 6e r 01Àn ðe r 0Àa ÁD T Þðe Àa ÁD T Þ;e P e r 0under compression1;e P e r 0under tension8<:ð6Þwhere D ðe r ;D T Þrepresents the thermal damage with respect to the thermal strains.e r 0is the strain at peak-strength;n ð¼S r =S Þis the coeﬃcient of residual strength for a RVE,S and S r are the peak-strength and residual strength,respectively.Under compression,n is less than 1but greater than 0.Under tension,n is equal to 0.When the strain e becomes smaller than or equal to e r 0,the RVE is undamaged and intact,and D ¼0.When the strain e is larger than e r 0,and under a compressive state,the RVE is damaged,i.e.D >0,and damage variable shall be calculated by the residual strength.Under a tensile state,the RVE is fully damaged and does not sustain any load,and D ¼1.The behavior for a given state of thermal induced damage can be represented by r ¼½1ÀD ðe r ;D T Þ ÁE 0Áðe r Àe thermal Þð7ÞHence,substituting Eq.(6)into Eq.(7),a mesoscopic nonlocal damage model,which can describe the com-plete thermal induced damage process,is expressed as:r ¼E 0Áðe Àa ÁD T Þ;e thermal 6e 6e r 0n ÁE 0Áðe r 0Àa ÁD T Þ;e P e r 0under compression0;e P e r 0under tension8<:ð8ÞIn order to simulate the thermal damage induced by thermal tensile or compressive stresses,a failure crite-rion,which can consider the eﬀects of both tension and compression,is necessary.In this study,the Mohr–Coulomb criterion with tension cutoﬀ[16]is chosen as the criterion of cracking:r 1À1þSin h r 2P S c if r 1P S c 1À1þSin h Á1ÀÁor r 26ÀS t if r 16S c 1À1þSin h 1ÀSin h Á1k ÀÁ8<:ð9Þwhere S c and S t are the uniaxial compressive strengthand tensile strength respectively,S t ¼Àk ÁS c ,and k is the ratio of tensile strength to compressive strength.h is the friction angle of the material.All these parameters can be obtained experimentally.r 1and r 2are the maximum and minimum principal stresses respectively.A compressive stress is positive,and a tensile stress is negative.Finally,a ﬁnite element program T-MFPA,incor-porating the above-mentioned MTED model and failurecriteria,was developed based on the Material Failure Process Analysis (MFPA)program [11,12],using a four-node isoperimetric element.2.3.Numerical specimensNumerical tests of ﬁve specimens (one circular spec-imen and four square specimens)using the T-MFPA program are reported in following sections.Let a i de-note the CTE of the inclusion and a m be the CTE of the matrix.The specimens were analyzed under a plain stress condition without external loading.Specimen no.1is a circular specimen comprising two diﬀerent homogeneous phase materials (matrix and in-clusion,see Fig.3a).It is numerically heated under a uniform temperature ﬁeld of 50°C,and free boundary conditions.The numerical thermal stresses determined from the proposed program are compared with those derived from the classical theory of thermoelasticity,from which the validity of the MTED model in an elastic and undamaged state can be justiﬁed.The me-chanical and geometrical properties of the phase mate-rials are listed in Table 1.In this case,the CTE of the inclusion is greater than that of the matrix.A homoge-neity index h ¼300is chosen so that the phase materials are basically homogeneous in nature.The numerical results are shown in the following section.Y.F.Fu et al./Cement &Concrete Composites 26(2004)99–111103In order to determine the eﬀects of material hetero-geneity,material strength,and CTE on the stress de-velopment and the process of thermal cracking around a single inclusion,four square specimens (Specimens no.2to no.5,see Fig.4)with diﬀerent thermal and me-chanical properties (see Table 2)are numerically stud-ied.Basically,the specimens can be classiﬁed into two groups.In Group 1(Specimens no.2and no.3),the CTE of the matrix is smaller than that of the inclusion.In Group 2(Specimens no.4and no.5),the CTE of matrix is larger than that of the inclusion.Within a group,the only variable is the mean strength of the in-clusion.The four specimens have the same homogeneity index h equal to 3,representing a high degree of heter-ogeneity.They are subjected to a uniform temperature increment from 20to 620°C at an incremental step of 10°C.3.Model validationFig.3b shows the comparison of the thermal stresses around the single inclusion of Specimen no.1calculated from the T-MFPA program,and from the analytical solutions (Eqs.(10)and (11))derived from the classical theory of thermo-elasticity [6,17].It is evident that under an elastic and undamaged state,an excellent agreement between the stresses ob-tained from the two diﬀerent approaches has been ob-tained.4.Thermal cracking history of square specimens Fig.5shows the eﬀect of thermal mismatch on the thermal induced damages and fracture processes of Specimen no.2(Group 1)and Specimen no.4(Group 2)due to increasing temperatures.Fig.6illustrates the inﬂuence of the mean strength of the inclusions on the crack development in each group.Detailed descriptions of crack formation of the specimens are shown below.4.1.Thermal cracking in composite of a i >a mIn the case of Specimen no.2,since the a i (CTE)of the inclusion is greater than that of the matrix ða m Þ,the incompatibility of thermal deformation at the interface between the matrix and the inclusion leads to the stress concentration around the inclusion (see Fig.5a(a)).The inclusion is under a statistically hydrostatic compres-sion,and the matrix is under a combination of com-pression and tension.When the temperature reaches 200°C,a few broken elements randomly occur (due to heterogeneity)in the high stress zone around the inclu-sion.With increasing temperatures,the number of the diﬀused damaged elements increases.The damaged ele-ments exist in both the high stress zone and in the low stress zone,but most of them are located near the for-Table 1Material properties of circular Specimen no.1ParameterValue Matrix Inclusion Heterogeneity index (h )300300Mean elastic modulus (MPa)60,000100,000Mean compressive strength (MPa)3060Poisson ratio0.250.20Coeﬃcient of thermal expansion (/°C) 1.0E )5 1.1E )5Temperature increment (°C)1010Tension cutoﬀ0.10.1Frictional angle (°)3030Diameter (mm)10020Number of elements31,4001256Fig.4.Numerical square specimen with single inclusion.Table 2Material properties of square Specimens no.2to no.5ParameterValue Matrix Inclusion Heterogeneity index (h )33Mean elastic modulus (MPa)60,000100,000Mean compressive strength (MPa)Specimen no.2200300Specimen no.3150Specimen no.4300Specimen no.5150Poisson ratio0.250.20Coeﬃcient of thermal expansion (/°C)Specimen no.2 1.0E )51.1E )5Specimen no.3 1.1E )5Specimen no.40.9E )5Specimen no.50.9E )5Temperature increment (°C)1010Tension cutoﬀ0.10.1Frictional angle (°)3030Dimension (mm)100Â100U 30Number of elements200Â2001412104Y.F.Fu et al./Cement &Concrete Composites 26(2004)99–111merly broken elements in the high stress zone (see Fig.5a(c)).When the temperature increases to 430°C,a macro-crack is formed ﬁrstly at the top-left area around the inclusion.At the same time,only a few of cracks nucleate far away from the high stress zone around the inclusion (see Fig.5a(d)).As the temperature further increases,the broken elements around the inclusion nucleate into several discontinuous macro-cracks (see Fig.5a(e)and (f)),and simultaneously corresponding tensile stress zones are formed at the tips of these cracks.Bridges are formed between the cracks due to the fact that many small cracks simultaneously grow at diﬀerent locations caused by the heterogeneity.This phenomenon is also described by Van Mier [19].As the temperature rises to 570°C,all these macro-cracks further propagate under the tensile stresses at their tips,followed by the occurrence of dispersed damaged elements in the frac-ture process zone.During the heating process,the macro-cracks are formed in the way that the discontin-uous cracks continue to grow and bridges are formed.The shapes of these cracks are irregular,rough and bi-furcate (see Fig.5a(e)–(h)).The macro-cracks formed along the radial direction around the inclusion can be called ‘‘radial cracks’’,which were also evident intheFig.5.(a)Thermal cracking of cement-based composite of Specimen no.2(inclusion diameter ¼30mm and a i ¼1:1Â10À6/°C).(b)Thermal cracking of cement-based composite of Specimen no.4(inclusion diameter ¼30mm and a i ¼0:9Â10À6/°C).Y.F.Fu et al./Cement &Concrete Composites 26(2004)99–111105experiments reported by Zhou et al.[18]and Golter-mann [5].It is also noted that when the main macro-cracks begin to propagate,the pace of minor crack develop-ment is slow down (see Fig.5a(g)and (h)).Fig.6a shows the thermal fracture process of the companion Specimen no.3with a lower mean strength ðr i3Þof the inclusion than that ðr i2Þin Specimen no.2.It is evident that the variation of the mean inclusion strength does not aﬀect the patterns of thermal damage initiation and propagation.4.2.Thermal cracking in composite of a i <a mIn the case of Specimen no.4,since the a i (CTE)of the inclusion is smaller than that of the matrix ða m Þ,azone of stress concentration also occurs around the in-clusion.The inclusion is stressed under tension and the matrix is under a combination of tension and com-pression (see Fig.5b(a)).When the temperature reaches 160°C,a few of the damaged elements distribute dis-orderly inside the inclusion.With increasing tempera-tures,the number of broken elements grows,and a few of them occur in the stress concentration zone outside the inclusion (see Fig.5b(c)).When the temperature increases to 400°C,the broken elements at the interface between the matrix and the inclusion nucleate and form several small discontinuous cracks.As the temperature becomes further higher,the discontinuous cracks at the interface propagate gradually and coalesce with the stress transferring from the inclusion and the matrix nearby the inclusion to the tips of the cracks (seeFig.Fig.6.(a)Thermal cracking processes of specimens in Group 1.(b)Thermal cracking processes of specimens in Group 2.106Y.F.Fu et al./Cement &Concrete Composites 26(2004)99–1115b(e)–(g)).Eventually,after the temperature has reached 620°C,most of all the elements around the interface between the matrix and the inclusion are broken and a nearly close circular macro-crack is formed at the in-terface.The high stress distributing inside the inclusion is transferred into the crack tips.This kind of crack is called‘‘tangential crack’’,which is also observed in the experiments by Zhou et al.[18]and Goltermann[5].Fig.6b demonstrates the thermal fracture process of the companion Specimen no.5with a lower mean strengthðr i5Þinclusion than thatðr i4Þin Specimen no.4. Although the thermal damage initiation and crack propagation of the two specimens are similar,the number of the broken elements and the kinds of cracks at each temperature level are diﬀerent.At a lower tem-perature,more elements in the inclusion of Specimen no. 5are damaged than those in Specimen no.4(see Fig. 6b(a)and(a0)).When the temperature reaches360°C, the macro-cracks pass through partly or wholly the in-clusion,and high stresses previously distributed around the inclusion are transferred into the tips of these cracks (as shown in Fig.6b(b0)).With increasing temperatures, these discontinuous cracks nucleate and coalesce with the redistributing stressﬁeld(as shown in Fig.6b(c0)and (d0)).This kind of crack occurred inside the inclusion is called‘‘inclusion crack’’.5.Thermal stressﬁelds of square specimens5.1.Eﬀect of thermal mismatchAlthough the four specimens are subjected to uniform temperature changes,local stress concentration occurs around the inclusion due to the thermal mismatch be-tween the matrix and the inclusion.When the CTE of the inclusion is greater than that of the matrix,the inclusion in Specimen no.2is stressed under a state of statistically hydrostatic compression due to the restriction from the matrix,and the matrix is under a general bi-axial state of stresses(tensile/com-pressive and shear stresses)due to the outward expan-sion from the inclusion.The distribution of maximum and minimum principal stresses and the maximum shear stress along the mid-section of Specimen no.2can be shown in Fig.7a(a).Although the maximum and mini-mum principal stresses in the inclusion are high,the maximum shear stress is much smaller so that few ele-ments with lower strength in this area reach their failure strength.The absence of tensile stresses in the inclusion delays the attainment of the Mohr–Coulomb with ten-sion cutoﬀfailure criterion.Unless the inclusion is ab-normally weak in compression,the strength of inclusion has no eﬀect on damage initiation(see Fig.6a).As a result,most of the diﬀused damages distribute in the high stress zone of the matrix around the inclusion for Group1specimens.With increasing temperatures,these broken elements nucleate and form several discontinu-ous cracks due to the stress redistribution at the crack tips.Since the minimum principal stress is nearly per-pendicular to the radial direction of the inclusion and is in tension,these cracks are developed in the manner of radial cracks in the matrix.When the CTE of the inclusion is smaller than that of the matrix,the inclusion in Specimen no.4is stressed under bi-axial tension,and the matrix remains in a state of compressive/tensile and shear stresses(see Fig.7b). Since a bi-axial tension leads to early attainment of the failure criterion,it is not surprised that the initiation of damage takes place only in the inclusion of Group2 specimens.In such a case,the strength of the inclusion has considerable eﬀects on the crack formation.That is, a weaker inclusion will have damage initiated at a lower temperature and grow more rapidly at high tempera-tures(see Fig.6b).The minimum principal stress in the matrix is parallel to the radius direction of the inclusion and is in tension,so that the main cracks propagate in the manner of tangential cracks at the matrix–inclusion interfacial region.5.2.Eﬀect of heterogeneity at meso-scaleThe thermal stressﬁelds are shown in Figs.4–6.The bright color indicates the higher stress,and vice versa.It is found that the points with diﬀerent scale colors exist in a same zone.It means that there are existing points subjected to diﬀerent stresses due to the heterogeneity at meso-scale in such zone,where the stressﬁeld is statis-tically uniform at macro-scale.The ratio of the local stress to the local strength is a very important parameter which can be used to decide whether or not an element fails.The eﬀect of the heterogeneity at meso-scale can be reﬂected by the stressﬂuctuation shown in Fig.7.In comparison with the results from Fig.3,the curves of stress distribution along the mid-section E–E in Speci-mens no.2to no.5before crack initiating are charac-terized by an irregular variation of stress values(see Fig. 7a and b).Such a strong thermal stressﬂuctuation in a heterogeneous composite,which can be quantitatively identiﬁed in our numerical study,is diﬃcult to be de-termined by experiments.Taking into account of the material heterogeneity, the failure of a material is dependent both on the in-duced stress level and on the strength itself.An element subjected to high stress may not break due to the fact that this element has higher strength;whereas an ele-ment subjected to low stress may break because of its low strength.These kinds of failure are deﬁnitely dif-ferent,since their released energies are diﬀerent.Con-sequently,some RVE can still remain un-fractured in a zone of high stress,if these elements have higherY.F.Fu et al./Cement&Concrete Composites26(2004)99–111107。

材料科学与工程专业英语课后习题答案unit1-7

Materials science材料科学Stone age石器时代Naked eye肉眼Bronze age铜器时代Optical property光学性能Integrated circuit集成电路Mechanical strength机械强度Thermal conductivity导热“Materials science” involves investigating the relationships that exist between the structures and properties of materials. In contrast ,”materials engineering “is ,on the basis of there structure property correlations ,designing or engineering the structure of a material that produce a predetermined set of properties。

，材料工程是根据材料的结构和性质的关系来设计或操纵材料的结构以求制造出一系列可预定的性质。

从功能方面来说，材料科学家的作用是发展或合成新的材料V irtually all important properties of solid materials may be grouped into six different categories: mechanical, electrical, thermal, magnetic, optical ,and deteriorative。

固体材料的所有重要的性质可以分成六个不同的种类，机械性能、电性能、热性能、磁性能、光性能和内耗。

In addition to structure and properties , two other important components are involved in the sciences and engineering of materials , namely“processing”and“performance”.除了组织性能之外，另外两个重要的性质也包括在材料科学和工程之中，即“加工”和“特性”The more familiar an engineer or scientist is with the various characteristics and structure-property relationship，as well as processing techniques of material，the more proficient and confident he or she will be to make judicious materials choices based on these criteria。

The Properties of Carbon Fibers and Composites

The Properties of Carbon Fibers andCompositesCarbon fibers and composites are a type of material that have become increasingly popular in recent years due to their unique properties. These materials are made from carbon fibers that are bonded together with a polymer matrix, resulting in an incredibly strong and lightweight material. In this article, we will explore the properties of carbon fibers and composites in more detail.1. High Strength and StiffnessOne of the most significant properties of carbon fibers and composites is their high strength and stiffness. Carbon fibers themselves are incredibly strong, with tensile strength of up to 700 ksi. When bonded together with a polymer matrix, the resulting composite material is even stronger, making it an ideal choice for applications where strength and stiffness are critical, such as aerospace and automotive industries.2. LightweightCarbon fibers and composites are also incredibly lightweight. Carbon fibers have a specific gravity of 1.75 g/cm3, which is much lower than other high-strength materials such as steel or aluminum. When combined with a polymer matrix, the resulting material is even lighter, making it a popular choice for applications where weight is a concern, such as in sports equipment or in the construction of airplanes.3. High Thermal ConductivityAnother property of carbon fibers and composites is their high thermal conductivity. Carbon fibers are excellent conductors of heat, allowing them to quickly dissipate any heat generated within the material. This property makes carbon fiber composites an ideal choice for applications where heat dissipation is critical, such as in electronic equipment or in the construction of heat sinks.4. Low Thermal ExpansionCarbon fibers and composites also have a low coefficient of thermal expansion. This property means that the material remains dimensionally stable even when subjected to changes in temperature. This characteristic makes carbon fiber composites ideal for use in applications where dimensional stability is important, such as in the construction of satellites or in precision instruments.5. Corrosion ResistanceCarbon fibers and composites are highly resistant to corrosion. Unlike metals, which are prone to rust and other forms of corrosion, carbon fibers and composites can withstand exposure to harsh chemicals and other corrosive substances. This property makes carbon fiber composites an ideal choice for use in harsh environments, such as offshore oil rigs or chemical processing plants.In conclusion, carbon fibers and composites are a type of material that possess unique properties that make them an ideal choice for a variety of applications. Their high strength and stiffness, lightweight nature, high thermal conductivity, low coefficient of thermal expansion, and corrosion resistance make them a popular choice for use in industries such as aerospace, automotive, sports equipment, electronics, and more. With ongoing research and development, it is likely that we will see even more innovations in carbon fiber and composite technology in the future.。

热能专业的英语书

热能专业的英语书Thermal energy, as an essential branch of engineering, plays a pivotal role in various industries such as power generation, automotive, and aerospace. The study of thisfield requires a solid understanding of thermodynamics, heat transfer, and fluid dynamics. A specialized textbook inEnglish for students of thermal energy would typically cover the following topics:1. Introduction to Thermal Sciences: This section would introduce the fundamental concepts of thermal energy,including temperature, heat, and the laws of thermodynamics.2. Thermodynamics: A detailed exploration of the first, second, and third laws of thermodynamics, including the principles of energy conservation and entropy.3. Heat Transfer: This chapter would delve into the three modes of heat transfer: conduction, convection, and radiation, along with the mathematical models used to describe these processes.4. Fluid Mechanics: An examination of the behavior of fluids, including fluid statics, dynamics, and the principles governing fluid flow.5. Thermal Systems: A study of various thermal systems such as heat engines, refrigerators, and heat pumps, with anemphasis on their efficiency and performance.6. Combustion and Energy Conversion: This section would cover the principles of combustion, energy conversion processes, and the environmental impact of energy production.7. Renewable Energy Sources: An overview of renewable energy technologies such as solar thermal, geothermal, and bioenergy, and their integration into thermal systems.8. Advanced Topics: This part of the book could include advanced topics like thermodynamic cycles, thermodynamic properties of substances, and computational methods in thermal engineering.9. Case Studies: Real-world applications and case studies to illustrate the practical implementation of thermal energy principles.10. Problem Sets and Solutions: A collection of problems with detailed solutions to reinforce understanding and provide practical exercises.11. Glossary: A comprehensive glossary of terms used in the field of thermal energy.12. Appendices: Additional resources such as tables of thermodynamic properties, conversion factors, and mathematical formulas.This textbook would be an invaluable resource forstudents, educators, and professionals in the field, providing a comprehensive and in-depth exploration of thermal energy principles and their applications.。

Study of the properties of phonons in solids

Study of the properties of phonons insolidsIntroduction:Phonon is an important quasiparticle responsible for the transmission of sound and heat in solids. A thorough understanding of phonon properties can help us design new materials with desired thermal and mechanical properties. In this article, we will delve into the study of phonons in solids and explore their properties in detail.What are phonons?Phonons are quasiparticles that represent the collective motion of atoms in a solid lattice. They are responsible for the transmission of sound and heat in solids. When a disturbance, such as a temperature gradient or a mechanical force, is applied to a solid, it creates a vibration in the lattice. These vibrations are quantized and can be thought of as packets of energy that propagate through the lattice. These packets of energy are known as phonons.Phonon dispersion relation:The properties of phonons can be studied using the phonon dispersion relation, which describes the relationship between the frequency and wave vector of the phonons. The phonon dispersion relation can be obtained by solving the equations of motion for the atoms in the solid lattice. The phonon dispersion relation is an important tool for understanding the thermal and mechanical properties of solids.Phonon modes:The phonons in a solid can be classified into different modes based on their polarization. The three main phonon modes are longitudinal, transverse, and optic phonons. Longitudinal phonons are vibrations that occur parallel to the direction of wave propagation. Transverse phonons are vibrations that occur perpendicular to the directionof wave propagation. Optic phonons are vibrations where there is a displacement of the charges in the crystal resulting in a change in the electrostatic energy.Phonon thermal conductivity:Thermal conductivity is an important property of materials that quantifies the transfer of heat through them. Phonons play a major role in the thermal conductivity of solids. The thermal conductivity of a solid can be calculated by considering the contribution of each phonon mode to the overall thermal conductivity. The contribution of each phonon mode depends on its frequency and the phonon scattering processes that occur due to the impurities present in the solid.Phonon scattering:Phonon scattering is the process by which phonons interact with other phonons or defects in the lattice. Phonon scattering can occur due to impurities, lattice defects, and thermal vibrations of atoms. These scattering processes can affect the phonon dispersion relation and the thermal conductivity of the solid. Understanding and controlling these scattering processes is important for designing materials with desired thermal and mechanical properties.Conclusion:In conclusion, the study of phonons in solids is an important field of research that has implications in a wide range of areas such as thermoelectric materials, heat transfer, and acoustic devices. Understanding the properties of phonons and their interaction with the lattice defects and impurities will help us design new materials with improved thermal and mechanical properties.。

TheScienceandEngineeringofMicroelectronicFabricati

The Science and Engineering of MicroelectronicFabrication 课后练习题含答案本文为 The Science and Engineering of Microelectronic Fabrication 的课后练习题，含有答案。

该课程旨在介绍微电子制造的基础知识及其相关工艺。

下面是该课程的练习题及解答。

Chapter 11. What is the general definition of microelectronic fabrication?Microelectronic fabrication is the process of manufacturing integrated circuits or microelectromechanical systems (MEMS) on a small scale using various techniques including photolithography, etching, and deposition.2. What is a typical size of an integrated circuit?A typical size of an integrated circuit is in the range of a few millimeters to a few centimeters.3. What is the importance of clean room environment in microelectronic fabrication?Clean room environment is important in microelectronic fabrication because the presence of contaminants can negatively affect the functionality and reliability of the fabricated devices. Contaminants can cause defects, short circuits, and other problems.4. Name three mn types of microelectronic fabrication processes.The three mn types of microelectronic fabrication processes are deposition, lithography, and etching.5. What is the function of a photomask in lithography?A photomask is a template used in lithography to transfer a pattern onto a substrate. The photomask contns the pattern, which is projected onto the substrate using a light source. The photomask determines the size and shape of the pattern that is transferred onto the substrate.Chapter 21. What is the difference between dry and wet etching?Dry etching is a process in which the material is removed from the substrate using a plasma etch. Wet etching is a process in which the material is removed from the substrate using a liquid etchant. Dry etching is more precise and less damaging to the substrate than wet etching, but it is also more expensive.2. What is the function of a mask in etching?A mask is used in etching to protect certn areas of the substrate from being etched. The mask is made from a material that is resistant to the etchant, and it is placed on the substrate before the etching process begins.3. What is the difference between isotropic and anisotropic etching?Isotropic etching removes material in all directions, whereas anisotropic etching removes material in a specific direction. Anisotropic etching is more precise than isotropic etching, but it is also more difficult to control.4. What are the advantages of plasma etching over wet etching?Plasma etching is more precise than wet etching and can be used to etch a wider range of materials. It is also less damaging to the substrate.5. Name two types of dry etching.The two mn types of dry etching are reactive ion etching (RIE) and deep reactive ion etching (DRIE).Chapter 31. What is the purpose of a chemical vapor deposition (CVD) process?The purpose of a chemical vapor deposition process is to deposit a thin film of material onto a substrate. The process involves introducing a gas or vapor mixture into a reaction chamber and allowing it to react on the surface of the substrate.2. What is the difference between physical vapor deposition (PVD) and chemical vapor deposition (CVD)?Physical vapor deposition is a process in which the material is evaporated in a vacuum and deposited onto the substrate. Chemical vapor deposition involves a chemical reaction between a gas or vapor mixture and the substrate.3. What is the function of a sputtering target in physical vapor deposition?A sputtering target is used in physical vapor deposition to generate a plasma of ions which are directed towards the substrate. The ions deposit the material onto the substrate to form a thin film.4. What is the purpose of ion implantation?Ion implantation is used to introduce dopants into a material in order to change its electrical properties. The process involves accelerating ions towards the substrate, where they penetrate the surface and become embedded in the material.5. What is the difference between epitaxy and doping?Epitaxy is the process of growing a thin film of material on a substrate. Doping involves introducing dopants into a material to change its electrical properties.。

小学上册L卷英语第三单元暑期作业(含答案)

小学上册英语第三单元暑期作业(含答案)英语试题一、综合题(本题有100小题，每小题1分，共100分.每小题不选、错误，均不给分)1.What is the term for a vehicle that travels on water?A. AirplaneB. CarC. BoatD. Train答案:C2.My brother is learning to _______ (动词). 他希望能 _______ (动词).3.In a chemical reaction, the mass of the products equals the mass of the _____.4. Wall stretches over ________ (长城绵延超过________) kilometers. The Grea5.What is the capital of Seychelles?A. VictoriaB. MahéC. PraslinD. La Digue答案:A6.What is the smallest continent?A. AsiaB. AfricaC. AustraliaD. Europe7.What is the capital of India?A. MumbaiB. DelhiC. KolkataD. Bangalore答案:B8.The chemical formula for ethanol is ________.9.The ancient Romans celebrated _______ to honor their gods. (节日)10.My sister has a strong interest in __________ (文学).11.He has a collection of _______ (邮票).12.I have a ___ of gum. (stick)13.My friend is a ______. He loves to travel the world.14.The _______ describes how much solute is dissolved in a solvent.15.The flowers are ________ in spring.16.The capital of Slovakia is __________.17.My sister likes _________ (洋娃娃) because she can dress them up in different _________ (衣服).18.What is the main purpose of a refrigerator?A. Cook foodB. Freeze foodC. Cool foodD. Warm food19.In chemistry, we study _______ which are made of atoms. (物质)20.The __________ tells us about the weather conditions. (预报)21.What do we call the large body of fresh water surrounded by land?A. OceanB. RiverC. LakeD. Pond22.The capital of South Korea is __________.23.My sister enjoys __________ (艺术创作).24.The chemical formula for sodium sulfate is ______.25. A _______ can help visualize the concept of thermal energy.26.The rabbit has powerful _______ (后腿) for jumping.27.The gas used in balloons is ______.28.I like to imagine what it would be like if my toys were real ________ (名词).29.We should ________ our toys.30.古代埃及的________ (writing) 系统是象形文字。

fabricationofhig...

J O U R NA L O F M AT E R I A L S S C I E N C E L E T T E R S16(1997)1600±1602Fabrication of high strength and high conductivity copper alloys by rod millingH.I.CHOI,K.Y.LEE,S.I.KWUNDepartment of Metallurgical Engineering,Korea University,Anam-dong,Sungbuk-ku,Seoul,KoreaCopper alloys are required to have both high strengthand high conductivity properties in order to conformto the recent trend towards smaller size,higherprecision and higher packing density in electric andelectronic parts.Among the strengthening mechan-isms of Cu alloys,grain re®nement strengthening isknown to be the most effective in increasing thestrength and minimizing the decrease in electricalconductivity[1±3].However,since most highstrength and high electrical conductivity materialscontain more than®ve alloying elements,it would bemuch more economic if we could®nd simple alloysystems that show equivalent or better properties.Itis well known that the solubility of Fe or Cr in Cu atlower temperatures is very limited[4].Mechanicalalloying[5]is a unique process in that it is anentirely solid state process,permitting extension ofsolubility beyond the equilibrium limit,®ne distribu-tion of insoluble phases and®ne-grain-size material.Rod milling was reported to produce alloyed oramorphous powders with high thermal stability andlow iron contamination[6].This research wasintended to develop,through rod milling,simplebinary Cu alloys possessing both high strength andhigh electrical conductivity.The vial and rods were coated with Cu tominimize contamination before mixtures of elemen-tal powders of Cu and Fe or Cr with purity higherthan99.9%were rod milled in argon atmosphere for400h.The alloyed powders were cold compacted ina Cu tube,vacuum sealed at5008C,then hotextruded at5008C with an extrusion ratio of16:1.After extrusion,specimens were heat treated at600,700and8008C for1h in vacuum to avoid oxidation,and subsequently furnace cooled.In order to examine the mechanical alloyingprocess,phase transformation and microstructuralchange in the alloys under investigation,the Cu±5vol%Fe composition was chosen as a prototypealloy composition.Even though both the completedisappearance of the Fe peak in the X-raydiffraction(XRD)pattern(Fig.1)and the plateauin the micro-hardness curve(Fig.2)after200hmilling indicated that the alloying process wascompleted after this time;the rod milling wasperformed for400h in order to ensure alloying. The optical microscopy also could not resolve any layered microstructure or individual grains in the powder after400h milling.The mean size of the alloyed powders was75ìm.Differential scanning calorimetry(DSC)analysis of the alloyed powders showed that the Fe started to precipitate out at about5408C.The mean grain size of the as-extruded specimen was200nm,and this grain size increased to about400nm even after heat treatment at8008C for1h,resulting in little decrease in0261-8028#1997Chapman&Hall 807060504030202θ (degrees)Intensity(arbitaryunits)(a)(b)(c)(d)Cu (220)Cu (200)Cu (111)Fe (110)Figure1XRD spectra of rod milled Cu±5vol%Fe powders as a function of milling time:(a)100,(b)200,(c)300and(d)400h.Hv(5g,15s)Milling time (h)Figure2Variation of Vickers hardness of rod-milled Cu±5vol%Fe powders as a function of milling time.1600tensile strength after heat treatment,as shown in Fig.3.The tensile strength of Cu±5vol %Fe alloy heat treated at 8008C for 1h followed by slow furnace cooling was as high as 746MPa,while the electrical conductivity was only 49%IACS (Inter-national Annealed Copper Standard).Based on these results for Cu±5vol %Fe alloy,the new alloy compositions of Cu±1vol %Cr and Cu±1vol %Fe were fabricated by the same procedures as before for higher electrical conductiv-ity.As a result of the addition of smaller amounts of alloying elements,the tensile strength decreased noticeably (Fig.3).On the other hand,the electrical conductivity increased signi®cantly in both alloys (Fig.4)compared to Cu±5vol %Fe alloy.Partial recrystallization after extrusion and partial grain growth of recrystallized grains after heat treatment at 7008C were observed in Cu±1vol %Cr alloy,as shown in Fig.5.In summary,this investigation showed that rod milling can be used in the fabrication of high strength and high electrical conductivity Cu binary alloys.In particular,the Cu±1vol %Cr alloy prepared by rod milling and subsequent hot extrusion followed by heat treatment at 7008C for 1h showed tensile strength of 620MPa,electrical conductivity of 70%IACS and elongation of11%.850800750700650600550500U T S (M P a )500600700800900400Annealing temperature (°C)Figure 3Effect of annealing temperature on tensile strength of the extruded bar.(j ),Cu±5%Fe;(d ),Cu±1%Fe;(m ),Cu±1%Cr.Figure 5Transmission electron micrographs of the Cu±1vol %Cr alloy.(a)as extruded and (b)after heat treatment at 7008C for 1h.500600700800900400Annealing temperature (°C)80706050403020C o n d u c t i v i t y (%I A C S )Figure 4Effect of annealing temperature on electrical conductivity of the extruded bar.(j ),Cu±1%Cr;(d ),Cu±1%Fe;(m ),Cu±5%Fe.1601AcknowledgementFinancial support from the Korean Ministry of Education through a research fund is greatly acknowledged.References1. A.ROT E N and A.RO S E N,Met.Trans.A16(1985)2073.2.J.H.M E N D E N H A L L,``Understanding copper alloys''(JohnWiley&Sons,Chichester,1980).3. B.FA R G E T T E,Met.Technol.6(1979)194.4.T. B.M A S S A L S K I(editor),``Binary phase diagram''(APDIC).5.J.S.B E N JA M I N,Met.Trans.A1(1970)2943.6.M.S.E L-E S K A N DA R A N Y,K.AO K I and K.S U Z U K I,J.Less Common Metals167(1990)113.Received12November1996and accepted13June19971602。

Effect of soil type and moisture content on ground

Effect of soil type and moisture content on groundheat pump performanceW.H.Leong a,V.R.Tarnawski b and A.Aittoma¨ki ca Hatch Associates Ltd,Non-Ferrous Business Unit,Energy and FluidMechanics Group,2800Speakman Drive,Mississauga,Ontario L5K2R7,Canadab Division of Engineering,Saint Mary’s University,Halifax,Nova Scotia B3H3C3,Canadac Institute of Energy and Process Engineering,Tampere University ofTechnology,Tampere,S-F33101FinlandThree different soils(sand,silty loam and silty clay)withﬁve different degrees ofsaturation(0,12.5,25,50and100%)were used in computer simulations.The performance ofa ground heat pump system was found to depend strongly on the moisture content and the soiltype(mineralogical composition).Alteration of soil moisture content from complete drynessto12.5%of saturation strongly inﬂuences the ground heat pump performance,and anydecrease of soil moisture in this range has a devastating effect on the coefﬁcient ofperformance(COP).Therefore,it is beneﬁcial to keep the soil moisture value as high aspossible above dry soil conditions.Soil moisture content above the quarter saturation stateleads to a much better heat pump performance.It was found,however,that the effect ofmoisture content variation above50%of saturation on ground heat pump performance isrelatively insigniﬁcant.᭧1998Elsevier Science Ltd and IIR.All rights reserved.(Keywords:thermal properties;ground heat pumps;heat exchangers)Effet du type et de l’humidite´du sol sur la performance des pompes a`chaleur a`capteurs enterre´sOn a utilise´trois types de sol diffe´rents(sable,glaise limoneuse et argile limoneuse)aveccinq degre´s de saturation(0,12,5,25,50et100%)pour des simulations par ordinateur.Lesauteurs ont montre´que la performance d’un syste`me utilisant une pompe a`chaleur de´pendaitfortement de l’humidite´et du type du sol(c’est a`dire sa composition mine´ralogique).L’augmentation de l’humidite´du sol(d’un taux d’humidite´de ze´ro a`taux de12,5%)inﬂuencefortement la performance de la pompe a`chaleur et toute diminution de l’humidite´du sol danscette zone exerce des effets extreˆmement ne´fastes sur le COP.Donc,le maintien de l’humidite´du sol au niveau le plus e´leve´au dessus des conditions de sol sec inﬂuence favorablement laperformance.Un taux d’humidite´au-dessus du seuil d’un sol25%sature´donne lieu a`uneperformance nettement ame´liore´e de la pompe a`chaleur.Cependant,on a montre´que lesvariations dans le taux d’humidite´au dessus du seuil d’un sol50%sature´ont exerce´des effetsrelativement insigniﬁcants sur la performance.᭧1998Elsevier Science Ltd and IIR.Allrights reserved..(Mots cle´s:proprie´te´thermique;pompes a`chaleur sol;e´changeur de chaleur)IntroductionIn ground heat pump applications,deposition or extrac-tion of thermal energy from the ground is accomplished by using a ground heat exchanger(GHE),whose operation induces a simultaneous heat and moisture ﬂow in the surrounding soil.The transfer of heat between the GHE and adjoining soil is primarily by heat conduction and to a certain degree by moisture migration.Therefore,it depends strongly on the soil type,temperature and moisture gradients.The entire process of heat extraction/deposition is a transient one, Int J.Refrig.Vol.21,No.8,pp.595–606,1998᭧1998Elsevier Science and IIRAll rights reserved.Printed in Great Britain0140–7007/98/$19.00+00PII:S0140-7007(98)00041-3595due to the weather-dependent ground surface boundary conditions and heating/cooling load.The soil thermal conductivity varies greatly with the soil type(texture, mineralogical composition),moisture content,dry bulk density,temperature,and soil air humidity.The soil moisture content in close vicinity to the GHE can be inﬂuenced by numerous factors,such as:soil structure, temperature gradient,moisture gradient,irrigation,and gravity effects.In particular,the temperature gradient in the soil surrounding the GHE plays an important role in the combined heat and moistureﬂow.When the soil temperature near the GHE is well above40ЊC,the effect of the moisture gradient is limited as compared to the temperature gradient,which may lead to a dry soil belt around the GHE behaving like an annular zone of insulation1.Moreover,structural and textural properties of the same soil sample can vary considerably with seasonal climatic conditions.Therefore,thorough knowledge of the intricate nature of soils and transport phenomena related to coupled heat and moistureﬂow in the ground is essential to both the design and the operation of ground heat pump systems.Due to the very complex character of the ground,the actual design of the GHE should be based on a detailed mathematical model of simultaneous heat and moistureﬂow in soils,an integrated heat pump model and reliable ground hydro-geological data.The parameters inﬂuencing the soil thermal properties are essential for testing the GHE length and carrying out a parametric analysis,which is very useful for estimating the long-term effects of GHE operation on the surrounding ground.The results of laboratory testing of soil samples(grain size,soil dry bulk density,water content,mineralogical composition) should also be available prior to the evaluation of soil thermal properties.A few papers dealing with the inﬂuence of soil types and moisture conditions on ground heat pump perfor-mance have been published in the last decade.Hailey et al.2analyzed the behavior of the thermal conductivity of soils adjacent to the GHE.It was found that soil moisture content was a dominant factor responsible for seasonal thermal conductivity variations.High heat rejection rates to the ground(cooling mode)had a detrimental impact on the soil thermal conductivity,leading to reduction of heat transfer.Drown and Den Braven3monitored for several seasons the effect of soil conditions and thermal conductivity on heat transfer in ground heat storage. Augmentation of the soil thermal conductivity by 0.17W(m K)¹1led to reduction of heat pump operating time by1.3%.Heat transfer in multi-layered ground (coarse sand,clay,ﬁne sand)with a vertical GHE was examined by Deng and Fedler4.A two-dimensional model of unsteady heat conduction in soils was used to simulate the ground temperature proﬁles.The model disregarded soil moisture migration and assumed the average thermal properties of homogeneous and isotropic596W.H.Leong etal.Figure1Ambient temperature and snow depth(Ottawa1987–88)vs timeFigure1Tempe´rature ambiante et profondeur de la neige(Ottawa1987–88)en fonction de la dure´esoils.The heat transfer rates were found to be discontin-uous between soil layers.The effectiveness of heat distribution in coarse andﬁne sandy soils was higher by 62%and27%,respectively,than in clayey soils.The above literature review shows,however,a lack of a general analysis on the applicability of common textural classes of soils and their thermal properties for use in ground heat pump systems.Experimental assess-ment of the above task would be very time consuming, expensive,error prone,and limited to conditions at a particular site.Therefore,a computer package for horizontal ground heat exchanger analysis,design and simulation(HG-HEADS5,6)has been used to examine the inﬂuence of three different standard soils on ground heat pump performance.Five different constant values of soil moisture content corresponding to0,12.5,25,50 and100%of saturation have been used in computer simulations.The above values have been kept constant throughout the soil domain and the simulation period. Evaluation of thermal characteristics of soilsThe thermal and hydraulic properties of soils are evaluated by a special subroutine developed for use in the HG-HEADS package.Evaluation of soil thermal conductivity is based on the enhanced model originally developed by de Vries7.The subroutine used has been extracted from the computer package TheHyProS8,9,and was modiﬁed to meet the isothermal phase change(soil freezing/thawing)requirements.An isothermal phase change process,used in HG-HEADS,allows the use of larger simulation time steps10.Numerous changes and enhancements to the original de Vries model have been made,and the most important additions are listed below.•The soil system can be made of up to26mineralo-gical components whose individual characteristics, such as mass fraction,thermal conductivity,density, shape and speciﬁc heat,must be known.The default option considersﬁve principal soil minerals(quartz, feldspar,calcite,clay-minerals,mica).•A general relationship for mineralogical composition of any natural soil has been introduced.•Soil water is assumed to be a continuous medium over a full moisture range(dryness to saturation).•The hydraulic soil water model of Campbell11is used for evaluation of water vapor migration in soil air pockets.•Unfrozen water content in freezing soils follows rela-tions published by Williams12and Anderson and Tice13.Effect of soil type and moisture content on GHE performance597Figure2Heating load of the building vs timeFigure2Charge thermique bu baˆtiment en fonction de la dure´e•The air shape factor follows a logarithmic function of soil moisture content.•Equations by Carslaw and Jeager 14are used for shape values of soil constituents.The TheHyProS package evaluates the thermal conductivity,speciﬁc heat and hydraulic properties of practically any soil for a full range of moisture content (dryness to saturation)and temperatures from ¹30to 95ЊC.The required input data is as follows:•selection of twelve standard soils (according to USDA)or user-deﬁned soil with known composition (i.e.,a combination of clay,silt,sand and gravel);•dry bulk density or porosity;•temperature;and•moisture content (in terms of volumetric or mass based).The remaining data (e.g.,mineral characteristics and shape values)are default values which may be over-ridden by the user.The predicted thermal conductivitiesof soils were compared with the experimental data by Kersten 15,using only default data.In general,agreeable results 8,9were puter simulationThe inﬂuence of soil types on the performance of a ground heat pump system has been studied using the HG-HEADS program.The software has a number of built-in design features,such as:•twelve standard soils;•nine conﬁgurations of the GHE,namely:•single layer in series and parallel,•double layer:series counterﬂow,parallel counter-ﬂow,parallel parallelﬂow,•triple layer:parallel parallelﬂow,•quadruple layer:series counterﬂow,parallel counter-ﬂow,parallel parallelﬂow;•sixteen standard pipes for GHE (inner and outer dia-meters,wall thermal conductivity);598W.H.Leong etal.Figure 3Thermal conductivity of sand,silty loam and silty clay vs temperature (dry,half-and fully saturated soils)Figure 3Conductivite´thermique de sable,glaise limoneuse et argile limoneuse selon la tempe ´rature (sols sec,a `moitie ´sature ´et comple `tement sature ´)•thirteen working ﬂuids (brine and antifreeze solu-tions with various concentrations);•manufacturer’s data for twelve heat pump models.The following parameters have been considered in computer simulations:soils:sand (r db ¼1480kg m ¹3,f ¼0.441,m qz ¼82.8%),silty loam (r db ¼1230kg m ¹3,f ¼0.536,m qz ¼44.4%),silty clay (r db ¼1200kg m ¹3,f ¼0.547,m qz ¼23.1%);soil moisture content:0;0.125v sat ,0.25v sat ,0.50v sat ,1.0v sat ,where v sat ¼1¹r db /r s ;Effect of soil type and moisture content on GHE performance599Figure 4Variation of sand temperature at GHE inlet vs time (dry,half-and fully saturated conditions)Figure 4Variation de la tempe´rature du sable a `l’entre ´e de l’e ´changeur de chaleur a `capteurs enterre ´s en fonction de la dure ´e soil domain:depth ϫwidth ¼5m ϫ0.25m;75triangular ﬁnite elements;61nodes;small ﬁnite elements are used in the upper part of the domain (2m),the rest of domain is discretized with larger elements;GHE data:single layer (serpentine arrangement),depth ¼1.0m,horizontal spacing ¼1.0m,length ¼500m,polyethylene PE 3408(31.75mm nominal pipe diameter);heat pump data:WX041by WaterFurnace Interna-tional Inc.,12kW nominal heating capacity,summer entering air tem-perature 24ЊC,winter entering air temperature 16ЊC;The ambient temperature,the snow depth at the ground surface and the heating load for the heating season corresponding to the winter of 1987–88are displayed in Figures 1and 2.Results and discussionDry soils generally do not exhibit thermal conductivity variation with temperature (Figure 3).The thermal conductivity evaluation is based on the mineralogical composition,dry bulk density and air content which is equal to soil porosity.The value of thermal conductivity for moist soils is considerably higher than for dry soils.This is a strong argument for maintaining the soil water content as high as possible above the dry state.Saturated and half-saturated soils display a noticeable change in the thermal conductivity,particularly below the freezing point (0ЊC).The superior thermal conductivity of sand is mainly due to its high quartz content.In the ﬁrst 1000hours of the heating season,sand temperature at the GHE inlet (Figure 4)drops rapidly.This is because the sensible heat is a main mode of heat transfer and the rates of temperature decrease depend on600W.H.Leong etal.Figure 5Variation of the brine temperature (GHE entrance/exit)vs time,for saturated sand,silty clay and silty loamFigure 5Variation de la tempe´rature de la saumure (entre ´e/sortie de l’e ´changeur de chaleur a `capteurs enterre ´s)en fonction de la dure ´e circulating ﬂuid:sodium chloride in water (20%by weight),freezing point ¹17ЊC (when the temperature of the circulating ﬂuid falls below the ﬂuid freezing point,the program issues a warning and the ﬂuid properties are evaluated using T f þ1ЊC),ﬂow rate ¼11gpm;meteorological data:Ottawa (1987–88);simulation period:15August 1987(0h)–14August 1988(8760h);heating season:26September (1008h)–8April (5688h).the sand moisture content.An erratic temperature variation and a high rate of temperature change are observed for completely dry sand.During a heat pump off-operation period,dry sand around the GHE recovers heat from a peripheral zone of the ground;hence,its temperature rises rapidly due to a very low speciﬁc heat as compared with moist sand.Once the freezing point of water is reached,a release of latent heat from the moist sand is utilized by the GHE until the freezing process is over.Therefore,there is no sign of a temperature drop below the freezing point until the total amount of latent heat is removed from the sand undergoing freezing.For dry sand,there is obviously no latent heat to be removed, and its temperature continues to decline sharply down to about¹17.5ЊC,which nearly corresponds to the end of the heating season.A similar trend has also been observed for two other soils,except that the rates of temperature decrease for the two soils are slightly higher and the lowest temperature of the soils is about¹19ЊC. Figure5displays variation of the brine temperature at the entrance and exit of the GHE for the saturated soils under investigation.The soil moisture content in the immediate vicinity of the GHE has a strong effect on the brine temperature.In theﬁrst1500hours of the heating season,brine temperature drops rapidly at a similar rate for all saturated soils,and then remains constant(e.g.,at the GHE exit:¹1.5,¹1.8and¹2.0ЊC for sand,silty loam and silty clay,respectively).During this time, extraction of sensible heat from the moist soil causes a high soil temperature drop.In the remaining part of the heating season all moist soils in the vicinity of the GHE experience freezing and their temperature remains constant.Moreover,the brine temperatures at the entrance and exit of the GHE buried in sand are always higher than those for silty loam and silty clay.This is due to a larger amount of heat being extracted from the sand. As far as the amount of heat extraction is concerned (Figure6),its largest value is observed for saturated sand,particularly in the initial part of the heating season, followed by silty loam and silty clay.A rapid decrease of heat extraction in theﬁrst1500hours of the heating season followed by an almost steady heat removal is thenEffect of soil type and moisture content on GHE performance601Figure6Heat extraction at the GHE vs time(dry,half-and fully saturated sand,silty loam and silty clay)Figure6Extraction de la chaleur de l’e´changeur de chaleur a`capteurs enterre´s en fonction de la dure´e pour des sols(sable,glaise limoneuse et argile limoneuse)sature´s en fonction de la dure´eobserved.This behavior is due to the freezing of soils,as previously described.Half-saturated soils show a very similar change of heat extraction with time to that observed for saturated soils.For the steady state period,the heat extraction for the half-saturated sand is about 2%lower than for the saturated sand.Dry soils experience a much more rapid decrease of heat extraction than moist soils.This is caused by a very low heat capacity of the soil and an absence of the freezing process.For moist soils at 50and 100%of saturation,the ratios of heating load demand to heat pump capacity are approximately the same,with only a few days when supplemental heating is required (Figure 7),i.e.,when the ratio is greater than one.Any decrease of soil moisture content below half saturation leads to an increase in the number of days required for supplemental heating.For example,dry soils exhibit a sharp increase in that ratio up to a value of about 5.5.This indicates that dry soils must not be used for ground heat pump applications.Furthermore,soil moisture content is indeed one of the most controlling factors inﬂuencing ground heat pump performance.Its value should be between full saturation and half saturation.Moist sand at half and full saturation shows a slightly better heat pump COP than moist silty loam or silty clay (Figure 8);the COPs for the last two soils are almost identical.The relative difference in the COP for sand moisture contents corresponding to the saturated and the half-saturated state is about 1.5%.Further decrease of soil moisture content (i.e.0.25v sat and 0.125v sat )results in more signiﬁcant drops in the COP.For dry sand a sharp decline of the heat pump COP is observed;it drops as much as 35%with respect to the COP for saturated sand.Dry silty loam and silty clay show a much lower COP with respect to dry sand.The more valuable results602W.H.Leong etal.Figure 7Ratio of heating load to heat pump capacity vs time,for sand,silty loam and silty clay at dry,half-and fully saturated conditionsFigure 7Relation entre la charge thermique et le rendement de lma pompe a`chaleur en fonction de la dure ´e pour des types de sol diffe´rents (sable,glaise limoneuse et argile limoneuse)avec des degre ´s de saturation diffe ´rentes (moitie ´ou comple `tement sature ´)of sand are due to its much higher quartz content and its superior thermal conductivity even in the completely dry state.These results prove again that dry soils must not be used for ground heat pump applications as their use leads to a sharp decrease and low values of the COP. Almost steady values of brine temperature,heat extraction rate and COP for all soils at half and full saturation were observed(Figures5,6and8)in the last two-thirds of the heating season from2616to5688h. Therefore,the average values of the above quantities over that duration were compared against theﬁve different degrees of soil saturation.Figure9displays the variation of the average heat extraction rate from the ground vs soil degree of saturation.A very sharp increase of the heat extraction rate was obtained for all soils between0and12.5%of saturation,followed by a moderate increase between12.5and25%of saturation. The heat extraction rate between50and100%of saturation shows much better results and a relatively small variation.In all cases sand shows the best performance,followed by silty loam and silty clay.The variation of the average COP vs the degree of soil saturation(Figure10)shows a very similar trend.The average brine temperature at the exit of the GHE also rises with an increase of the degree of saturation (Figure11).The highest brine return temperatures are obtained for sand at all degrees of saturation,which can be explained in terms of the largest amount of heat extracted by the ground heat exchanger.For all soils under investigation,the average heat extraction,the COP and the brine temperatures are similar at fully saturated soil conditions.These results may imply that at fully saturated conditions these soils are equally suitable for ground heat pump applications.It is worth knowing,however,that the use of silty loam and silty clay is limited due to their high sensitivity to frost heave. Conclusions(1)The performance of a ground heat pump system wasfound to depend strongly on the moisture contentEffect of soil type and moisture content on GHE performance603Figure8Variation of the coefﬁcient of performance vs time,for dry and half-saturated soils604W.H.Leong et al.Figure9Variation of the average heat extraction rate(2616–5688h)from the ground vs soil degree of saturation Figure9Variation de l’extraction de chaleur moyenne(2616–5688h)du sol en fonction du degre´de saturation du solFigure10Variation of the average COP(2616–5688h)vs soil degree of saturationFigure10Variation du COP moyen(2616–5688h)en fonction de la saturation du soland the soil type(mineralogical composition).Dry soils show a very sharp decline of the COP—it is lower by up to35%with respect to saturated condi-tions.Alteration of soil moisture content from12.5% of saturation to complete dryness strongly decreases the ground heat pump performance,and any reduc-tion of soil moisture within this range has a devastat-ing effect on the COP.Therefore,it is beneﬁcial to keep the soil moisture value as high as possible above dry soil conditions.The best performance of the ground heat pump was obtained for sand at all degrees of saturation,as compared to silty loam and silty clay.(2)Soil moisture content above25%of saturation leadsto generally better heat pump performance.It was found,however,that the effect of moisture content variation above the half-saturated state on ground heat pump performance is relatively insigniﬁcant.The difference in the COP for sand moisture con-tents corresponding to50and100%of saturation is only about1.5%.(3)The freezing process of saturated soils releases sucha large amount of latent heat that,for the heat pumpsystem considered,it was not fully completed during the heating season.The freezing of soil around theground heat exchanger produces almost constant values of the brine temperature,heat extraction, and the COP.(4)The amount of heat extracted from the ground ishighest for sand,followed by silty loam and silty clay which both exhibit very similar results.As the saturation approaches100%,however,the amount of heat extracted from different soils is approxi-mately the same.(5)For saturated and half-saturated soils,the ratio of theheating load to the heat pump capacity is below unity for nearly all of the heating season.Therefore there is almost no need for supplemental heating.Reduc-tion of the soil moisture content from half saturation to dryness leads to an increasing demand for supple-mental heating.AcknowledgementsThe authors express their appreciation and thanks to the National Sciences and Engineering Research Council of Canada for providingfundstocarryout thisstudy,andtoMr Sam Mahmoody,an undergraduate student,for assisting in computer simulations and preparation ofﬁgures.Effect of soil type and moisture content on GHE performance605Figure11Variation of the average brine temperature(2616–5688h)at the GHE inlet vs soil degree of saturationFigure11Variation de la tempe´rature moyenne de la saumure(2616–5688h)a`l’entre´e de la pompe a`chaleur a`capteurs enterre´s en fonction du degre´de saturation du solReferences1.Hartley,J.G.,Black,W.Z.,Transient simultaneous heatand moisture transfer in moist unsaturated soils.Transac-tions of ASME.(1981)103(5),376–382.2.Hailey,S.M,Kast,T.P.,Drown,D.C.,Thermal conduc-tivity and soil conditions heat transfer effects on ground source heat pumps.Proceedings of the16th Annual Conference of Solar Energy Society of Canada,Halifax, Nova Scotia,June(1990),pp.317–322.3.Drown,D.C.,Den Braven,K.R.,Effect of soil conditionsand thermal conductivity on heat transfer in ground source heat pumps.Proceedings of the ASME JSES KSES Inter-national Solar Energy Conference,Maui,Hawaii,5–9 April(1992)4.Deng,Y.,Fedler, C.B.,Multi-layered soil effects onvertical ground-coupled heat pump design.Transactions of ASAE.(1992)35(2),687–6945.Tarnawski,V.R.,Leong,W.H.,Computer Simulation ofGround Coupled Heat Pump Systems.National Research Council of Canada,Ottawa,Contract No.CS944–9–4104 (1990)6.Tarnawski,V.R.,Leong,W.H.,Computer analysis,design and simulation of horizontal ground heat exchan-gers.International Journal of Energy Research,199317, 467–4777.de Vries,D.A.,Heat transfer in soils.In Heat and MassTransfer in the Biosphere,ed.D.A.de Vries and H.Afgan.John Wiley&Sons,New York(1975)pp.5–288.Tarnawski,V.R.,Wagner, B.,A new computerizedapproach to estimating the thermal properties of unfrozen soils.Canadian Geotechnical Journal.(1992).29,714–720 9.Tarnawski,V.R.,Wagner,B.,Modeling the thermalconductivity of frozen soils.Cold Regions Science and Technology.(1993)22,19–3110.Hromadka,T.V.II,Guymon,G.L.,Berg,R.L.,Someapproaches to modeling phase change in freezing soils.Cold Regions Science and Technology.(1981)4, 137–14511.Campbell,G.S.,Soil Physics with Basic:Transportmodels for soil–plant systems.Elsevier,New York (1985)12.Williams,P.J.,Properties and behavior of freezing soils.Norwegian Geotechnical Institute,Paper72(1967)13.Anderson,D.M.,Tice,A.R.,Predicting unfrozen watercontents in frozen soils from surface area measurements.Highway Research Record.(1972)393,12–1814.Carslaw,H.S.and Jeager,J.C.,Conduction of Heat inSolids,2nd edn.Oxford University Press,London(1986) 15.Kersten,M.S.,Laboratory research for the determinationof the thermal properties of soil.Engineering Experiment Station,University of Minnesota(1949)606W.H.Leong et al.。

聚氨酯保温材料研究进展

综述CHINA SYNTHETIC RESIN AND PLASTICS合成树脂及塑料， 2023， 40（4）： 72DOI：10.19825/j.issn.1002-1396.2023.04.15我国每年新增建筑面积约20 亿m2，其中，95%为高能耗建筑，需要采取节能措施，最简单的节能措施是使用外墙保温材料。

导热系数不大于0.12 W/（m·K）的材料称为保温材料［1］。

建筑上常用的保温材料主要有无机保温材料、发泡聚苯乙烯、聚氨酯（PU）及复合型材料，其中，PU保温材料以其良好的保温效果和阻燃性能，近年来得到快速发展。

本文综述了PU保温材料的研究进展。

1 生物基PUAcuña等［2］制备了生物质蓖麻油基硬质PU泡沫（RPUF）。

两种生物基RPUF均含有蓖麻油改性多元醇，一种是二乙醇胺改性蓖麻油的多元醇（BIO1），另一种是用苯膦酸改性BIO1的环氧化多元醇（BIO2），研究了可膨胀石墨（EG）和氧化石墨（GO）总掺入量为6%（w）的RPUF的蜂窝结构、热性能、阻燃性能和力学性能。

结果表明，GO促进了EG的分散，降低了泡沫的蜂窝尺寸；GO提高了RPUF的隔热性能和阻燃性能；RPUF/BIO2/EG/ GO阻燃等级为UL 94 V-0级，而RPUF/BIO2/EG仅为UL 94 V-2级；与RPUF/BIO1相比，RPUF/BIO2/ EG/GO的热释放速率（HRR）、总热释放量（THR）和总烟生成量分别降低了54%，24%，15%；与RPUF/BIO2相比，RPUF/BIO2/EG/GO的HRR和THR分别降低了46%，6%；RPUF/BIO1的压缩强度为0.07 MPa，RPUF/BIO2/EG/GO和RPUF/BIO2/EG 的压缩强度增加到0.11 MPa。

因此，可通过加入天然碳材料来开发生物基阻燃RPUF作为防火保温聚氨酯保温材料研究进展张萌（内蒙古交通职业技术学院，内蒙古赤峰 024005）摘要：介绍了聚氨酯（PU）保温系数的预测方法及影响因素研究进展。

高分子材料工程专业英语第二版课文翻译(基本全了

A 高分子化学和高分子物理UNIT 1 What are Polymer?第一单元什么是高聚物？What are polymers? For one thing, they are complex and giant molecules and are different from low molecular weight compounds like, say, common salt. To contrast the difference, the molecular weight of common salt is only 58.5, while that of a polymer can be as high as several hundred thousand, even more than thousand thousands. These big molecules or ‘macro-molecules’ are made up of much smaller molecules, can be of one or more chemical compounds. To illustrate, imagine that a set of rings has the same size and is made of the same material. When these things are interlinked, the chain formed can be considered as representing a polymer from molecules of the same compound. Alternatively, individual rings could be of different sizes and materials, and interlinked to represent a polymer from molecules of different compounds.什么是高聚物？首先，他们是合成物和大分子，而且不同于低分子化合物，譬如说普通的盐。