AMC10B试题及答案
M美国数学竞赛 AMC B 试题及答案解析

2003 AMC 10B1、Which of the following is the same asSolution2、Al gets the disease algebritis and must take one green pill and one pink pill each day for two weeks. A green pill costs more than a pink pill, and Al’s pillscost a total of for the two weeks. How much does one green pill cost?Solution3、The sum of 5 consecutive even integers is less than the sum of the ?rstconsecutive odd counting numbers. What is the smallest of the even integers?Solution4、Rose fills each of the rectangular regions of her rectangular flower bed witha different type of flower. The lengths, in feet, of the rectangular regions in her flower bed are as shown in the ?gure. She plants one flower per square foot in each region. Asters cost 1 each, begonias 1.50 each, cannas 2 each, dahlias2.50 each, and Easter lilies 3 each. What is the least possible cost, in dollars,for her garden?Solution5、Moe uses a mower to cut his rectangular -foot by -foot lawn. The swathhe cuts is inches wide, but he overlaps each cut by inches to make sure thatno grass is missed. He walks at the rate of feet per hour while pushing themower. Which of the following is closest to the number of hours it will take Moe to mow his lawn?Solution.6、Many television screens are rectangles that are measured by the length of their diagonals. The ratio of the horizontal length to the height in a standard television screen is . The horizontal length of a “-inch” television screen isclosest, in inches, to which of the following?Solution7、The symbolism denotes the largest integer not exceeding . For example., and . ComputeSolution.8、The second and fourth terms of a geometric sequence are and . Which ofthe following is a possible first term?Solution9、Find the value of that satisfies the equationSolution10、Nebraska, the home of the AMC, changed its license plate scheme. Each old license plate consisted of a letter followed by four digits. Each new license plate consists of three letters followed by three digits. By how many times is the number of possible license plates increased?Solution11、A line with slope intersects a line with slope at the point . What isthe distance between the -intercepts of these two lines?Solution12、Al, Betty, and Clare split among them to be invested in different ways.Each begins with a different amount. At the end of one year they have a total of. Betty and Clare have both doubled their money, whereas Al has managedto lose . What was Al’s original portion?Solution.13、Let denote the sum of the digits of the positive integer . For example,and . For how many two-digit values of is?Solution14、Given that , where both and are positive integers, find thesmallest possible value for .Solution15、There are players in a singles tennis tournament. The tournament issingle elimination, meaning that a player who loses a match is eliminated. In the first round, the strongest players are given a bye, and the remainingplayers are paired off to play. After each round, the remaining players play in the next round. The match continues until only one player remains unbeaten. The total number of matches played isSolution16、A restaurant offers three desserts, and exactly twice as many appetizers as main courses. A dinner consists of an appetizer, a main course, and a dessert. What is the least number of main courses that the restaurant should offer so that a customer could have a different dinner each night in the year ?Solution.17、An ice cream cone consists of a sphere of vanilla ice cream and a right circular cone that has the same diameter as the sphere. If the ice cream melts, it will exactly ?ll the cone. Assume that the melted ice cream occupies of thevolume of the frozen ice cream. What is the ratio of the cone’s height to its radius?Solution18、What is the largest integer that is a divisor offor all positive even integers ?Solution19、Three semicircles of radius are constructed on diameter of a semicircleof radius . The centers of the small semicircles divide into four linesegments of equal length, as shown. What is the area of the shaded region that lies within the large semicircle but outside the smaller semicircles?Solution20、In rectangle , and . Points and are on so thatand . Lines and intersect at . Find the area of .Solution21、A bag contains two red beads and two green beads. You reach into the bag and pull out a bead, replacing it with a red bead regardless of the color you pulled out. What is the probability that all beads in the bag are red after three such replacements?Solution22、A clock chimes once at minutes past each hour and chimes on the houraccording to the hour. For example, at 1 PM there is one chime and at noon and midnight there are twelve chimes. Starting at 11:15 AM on February , ,on what date will the chime occur?Solution23、A regular octagon has an area of one square unit. What is thearea of the rectangle ?Solution24、The ?rst four terms in an arithmetic sequence are , , , and ,in that order. What is the ?fth term?Solution25、How many distinct four-digit numbers are divisible by and have as theirlast two digits?Solution。
2002 AMC 10B Problems

2002 AMC 10B Problems Problem 1 The ratio 200220032001632• is: (A )61 (B )31 (C )21 (D )32 (E )23 Problem 2For the nonzero numbers and define cb a abc c b a ++=),,(Find (2,4,6) . (A )1 (B )2 (C )4 (D )6 (E )24Problem 3The arithmetic mean of the nine numbers in the set {9,99,999,9999,…,999999999} is a 9-digit number M, all of whose digits are distinct. The number M does not contain the digit(A )0 (B )2 (C )4 (D )6 (E )8Problem 4What is the value of 14)23()14)(23(+--+-x x x x , when x=4 ?(A )0 (B )1 (C )10 (D )11 (E )12Problem 5Circles of radius and are externally tangent and are circumscribed by a third circle, as shown in the figure. Find the area of the shaded region.(A )π3 (B )π4 (C )π6 (D )π9 (E )π12Problem 6For how many positive integers is 232+-n n a prime number?(A )none (B )one (C )two (D )more than two, but finitely many (E )infinitely manyProblem 7 Let be a positive integer such that n 1713121+++ is an integer. Which of the following statements is not true?(A )2 divides n (B )3 divides n (C )6 divides n (D )7 divides n (E )n>84 Problem 8Suppose July of year N has five Mondays. Which of the following must occur five times in the August of year N ? (Note: Both months have 31 days.)(A )Monday (B )Tuesday (C )Wednesday (D )Thursday (E )Friday Problem 9Using the letters A,M,O,S and U , we can form five-letter "words". If these "words" are arranged in alphabetical order, then the "word" U S A M O occupies position(A )112 (B )113 (C )114 (D )115 (E )116 Problem 10Supposethat and are nonzero real numbers, and that the equation 02=++b ax x has solutions and . Then the pair (a,b) is(A )(-2,1) (B )(-1,2) (C )(1,-2) (D )(2,-1) (E )(4,4) Problem 11The product of three consecutive positive integers is times their sum. What is the sum of the squares?(A )50 (B )77 (C )110 (D )149 (E )194 Problem 12For which of the following values of does the equation 621--=--x k x x x have no solution for ?(A )1 (B )2 (C )3 (D )4 (E )5 Problem 13Find the value(s) of such that 032128=-+-x y xy is true for all values of .(A )32 (B )or 2341- (C )or 32-41- (D )23 (E )or 23-41- Problem 14The number is the square of a positive integer . In decimal representation,the sum of the digits of is (A )7 (B )14 (C )21 (D )28 (E )35Problem 15The positive integers A, B, A-B , and A+B are all prime numbers. The sum of these four primes is(A )even (B )divisible by 3 (C )divisible by 5 (D )divisible by 7 (E )prime Problem 16For how many integers is nn -20 the square of an integer? (A )1 (B )2 (C )3 (D )4 (E )10 Problem 17A regular octagon ABCDEFGH has sides of length two. Find the area of △ADG .(A )224+ (B )26+ (C )234+ (D )243+ (E )28+ Problem 18Four distinct circles are drawn in a plane. What is the maximum number of points where at least two of the circles intersect?(A )8 (B )9 (C )10 (D )12 (E )16Problem 19Suppose that is an arithmetic sequence with 100...10021=+++a a a and 200...200102101=+++a a a . What is the value of(A )0.0001 (B )0.001 (C )0.01 (D )0.1 (E )1Problem 20Let a, b, and c be real numbers such that a-7b+8c=4 and 8a+4b-c=7 Then 222c b a +- is(A )0 (B )1 (C )4 (D )7 (E )8Problem 21Andy's lawn has twice as much area as Beth's lawn and three times as much as Carlos' lawn. Carlos' lawn mower cuts half as fast as Beth's mower and one third as fast as Andy's mower. If they all start to mow their lawns at the same time, who will finish first?(A )Andy (B )Beth (C )Carlos (D )Andy and Carlos tie for first (E )All three tieProblem 22m =90º. Let M and N be the midpoints Let △XOY be a right-angled triangle with XOYof the legs OX and OY, respectively.Given XN=19 and YM=22, find XY .(A)24 (B)26 (C)28 (D)30 (E)32Problem 23Let be a sequence of integers such that and for all positive integers and Then is(A)45 (B)56 (C)67 (D)78 (E)89Problem 24Riders on a Ferris wheel travel in a circle in a vertical plane. A particular wheel has radius feet and revolves at the constant rate of one revolution per minute. How many seconds does it take a rider to travel from the bottom of the wheel to a point vertical feet above the bottom?(A)5 (B)6 (C)7.5 (D)10 (E)15Problem 25When is appended to a list of integers, the mean is increased by . When is appended to the enlarged list, the mean of the enlarged list is decreased by . How many integers were in the original list?(A)4 (B)5 (C)6 (D)7 (E)82002 AMC 10B Solution Problem 1 Problem 2 (C) Problem 3 The following problem is from both the 2002 AMC 12B #1 and 2002 AMC 10B #3, so both problems redirect to this page. We wish to find , or ,or. This does not have the digit 0, so the answer is (A) 0Problem 4 The following problem is from both the 2002 AMC 12B #2 and 2002 AMC 10B #4, so both problems redirect to this page. (D) 11Problem 5A line going through the centers of the two smaller circles also go through the diameter. The length of this line within the circle is 3+3+2+2=10 Because this is the length of the larger circle's diameter, the length of its radius is 5The area of the large circle is 25π, and the area of the two smaller circles is 9π+4π=13π. To find the area of the shaded region, subtract the area of the two smaller circles from the area of the large circle. 25π-13π=12π (E)Problem 6 The following problem is from both the 2002 AMC 12B #3 and 2002 AMC 10B #6, so both problems redirect to this page.Factoring, we get )1)(2(232--=+-n n n n . Either n-1 or n-2 is odd, and the other is even. Their product must yield an even number. The only prime that is even is 2, which is when is 3. The answer is one. (B) . Problem 7 The following problem is from both the 2002 AMC 12B #4 and 2002 AMC 10B #7, so both problems redirect to this page.Since 4241713121=++, 211424114241)14241(lim 0<+<+<+<∞→n n n From which it follows that 114241=+nand n=42. Thus the answer is Problem 8 The following problem is from both the 2002 AMC 12B #8 and 2002 AMC10B #8, so both problems redirect to this page.If there are five Mondays, there are only three possibilities for their dates: (1,8,15,22,29),(2,9,16,23,30) , and (3,10,17,24,31) .In the first case August starts on a Thursday, and there are five Thursdays, Fridays, and Saturdays in August.In the second case August starts on a Wednesday, and there are five Wednesdays, Thursdays, and Fridays in August.In the third case August starts on a Tuesday, and there are five Tuesdays, Wednesdays,and Thursdays in August. The only day of the week that is guaranteed to appear five times is therefore Thursday. D Problem 9 There are "words" beginning with each of the first four letters alphabetically. Fromthere, there are with as the first letter and each of the first three lettersalphabetically. After that, the next "word" is USAMO , hence our answeris . Problem 10 The following problem is from both the 2002 AMC 12B #6 and 2002 AMC 10B #10, so both problems redirect to this page.Solution 1Since 0)())((22=++=++-=--b ax x ab x b a x b x a x , it follows by comparing coefficients that –a-b=a and that ab=bSince b is nonzero, a=1, and -1-b=1, so b=-2 . Thus (a,b)=(1,-2) CSolution 2Another method is to use Vieta's formulas . The sum of the solutions to this polynomial is equal to the opposite of the coefficient, since the leading coefficient is 1; in other words, a+b=-a and the product of the solutions is equal to the constant term (i.e, a*b=b ). Since is nonzero, it follows that and therefore (from the first equation), b=-2a=-2 Problem 11 The following problem is from both the 2002 AMC 12B #7 and 2002 AMC 10B #11, so both problems redirect to this page.Let the three consecutive positive integers be a-1,a,a+1. so , a(a-1)(a+1)=24a , so (a-1)(a+1)=24, 24=4*6 , so a=5. Hence, the sum of the squares is 77654222=++ B. Problem 12The domain over which we solve the equation is .We can now cross-multiply to get rid of the fractions, we get (x-1)(x-6)=(x-k)(x-2)Simplifying that, we get 7x-6=(k+2)x-2k . Clearly for k=5 we get the equation -6=-10 which is never true. The answer is EFor other , one can solve for : k k x 26)5(-=-, hence kk x --=526 . We can easily verify that for none of the other four possible values of is this equal to or , hence there is a solution for in each of the other cases. Problem 13We have 8xy-12y+2x-3=4y(2x-3)+(2x-3)=(4y+1)(2x-3), as (4y+1)(2x-3)=0must be true for all , we must have , hence x=3/2 D .Problem 14Since, 756425364256425)2(585•=•=•=N .Combing the 's and 's gives us,This is 2048 with sixty-four, 's on the end. So, the sum of the digits of is 2+4+8=14 B Problem 15 The following problem is from both the 2002 AMC 12B #11 and 2002 AMC 10B #15, so both problems redirect to this page.Since A-B and A+B must have the same parity , and since there is only one even prime number, it follows that A-B and A+B are both odd. Thus one of A,B is odd and the other even. Since A+B>A>A-B>2, it follows that A (as a prime greater than 2) is odd. Thus B=2, and A-2, A, A+2 are consecutive odd primes. At least one of A-2, A, A+2 is divisible by 3, from which it follows that A-2=3 and A=5. The sum of these numbers is thus 17, a prime, so the answer is prime. E .Problem 16 The following problem is from both the 2002 AMC 12B #12 and 2002 AMC 10B #16, so both problems redirect to this page.Solution 1Let n n x -=202 , with (note that the solutions do not give any additionalsolutions for ). Then rewriting,12022+=x x n . Since , it follows thatdivides . Listing the factors of , we find that x=0,1,2,3 are the only 4 D solutions (respectively yielding n=0,10,16,18 ).Solution 2Forand the fraction is negative, for it is not defined, and forit is between 0 and 1. Thus we only need to examine and . For and we obviously get the squares and respectively.For prime the fraction will not be an integer, as the denominator will not contain the prime in the numerator.This leaves, and a quick substitution shows that out of these onlyn=16 and n=18 yield a square. Problem 17The area of the triangle ADG can be computed as 2AP DG • . We will now find DG and AP . Clearly, PFG is a right isosceles triangle with hypotenuse of length 2, hence PG=2. The same holds for triangle QED and its leg QD . The length of PQ is equal toFE=2 . Hence GD=2+22, and AP=PD=2+2. Then the area of ADG equals 2342)22)(222(2+=++=•AP DG C Problem 18 The following problem is from both the 2002 AMC 12B #14 and 2002 AMC 10B #18, so both problems redirect to this page.For any given pair of circles, they can intersect at most 2 times. Since there are pairs of circles, the maximum number of possible intersections is 6*2=12 . We can construct such a situation as below, so the answer is D .Problem 19Solution 1We should realize that the two equations are 100 terms apart, so by subtracting the two equations in a form likeWe can find the value of the common difference every hundred terms. But we forgot that it happens hundred times. So we have a divide the answer by hundred 100/100=1The answer yields us the common difference of every hundred terms. So you has to simply divide the answer by hundred again to find the common difference between one term 1/100=0.01 CSolution 2Adding the two given equations together givesNow, let the common difference be . Notice that, so we merely need to find to get the answer. The formula for an arithmetic sum is ))1(2(21-+n d a n where is the first term, is the number of terms, and is the common difference. Now we use this formula to find a closed form for the first given equation and the sum of the given equations. For the first equation, we have n=100 . Therefore, we have 50(2a 1+99d)=100, or *(1). For the sum of the equations (shown at the beginning of the solution) we have n=200, so 100(2a 1+199d)=300 or *(2) Now we have a system of equations in terms of and . Subtracting (1) from (2) eliminates, yielding 100d=1, and 100112=-=a a d C Solution 3Subtracting the 2 given equations yieldsNow express each a_n in terms of first term a_1 and common difference x between consecutive terms 100))99()199((...))2()102(())()101(())()100((11111111=+-++++-+++-++-+x a x a x a x a x a x a a x a Simplifying and canceling a_1 and x terms gives100x=1Problem 20a+8c=7b+4 and 8a-c=7-4b Squaring both,andare obtained. Adding the two equations and dividing by gives , soProblem 21 The following problem is from both the 2002 AMC 12B #17 and 2002 AMC 10B #21, so both problems redirect to this page.We say Andy's lawn has an area of . Beth's lawn thus has an area of2x , and Carlos's lawn has an area of 3x . We say Andy's lawn mower cuts at a speed of . Carlos's cuts at a speed of 3y , and Beth's cuts at a speed 32y . Each person's lawn is cut at a speed of rate area , so Andy's is cut in yx time, as is Carlos's. Beth's is cut in yx ⨯43, so the first one to finish is Beth. B . Problem 22 The following problem is from both the 2002 AMC 12B #20 and 2002 AMC 10B #22, so both problems redirect to this page.Let OM=x , ON=y . By the Pythagorean Theorem on △XON, MOYrespectively, ,19)2(222=+y x 22222)2(=+y xSumming these gives 8455522=+y x , so 16922=+y x . By the Pythagorean Theorem again, we have222)2()2(XY y x =+ so 26)169(4)(422==+=y x XY BProblem 23Solution 1First of all, write and in terms of a 2.can be represented by in different ways.Since both are equal toyou can set them equal to each other. 15212322+=+a a 32=a Substitute the value of back intoand substitute that intoSolution 2 Substituting into : . Since , . Therefore,and so on until. Adding the Left Hand Sides of all of these equations gives; adding the Right Hand Sides of these equations gives These two expressions must be equal; henceand and Substituting :Thus we have a general formula forand substituting m=12:Problem 24We can let this circle represent the ferris wheel with center and represent the desired point feet above the bottom. Draw a diagram like the one above. We find out is a triangle. That means and the ferris wheel has made of a revolution. Therefore, the time it takes to travel that much of a distance is of a minute, or seconds. The answer is . Alternatively, we could also say that is congruent to by SAS, so is 20, and is equilateral, andProblem 25 Let be the sum of the integers and be the number of elements in the list. Then2002 AMC10Bwe get the equations and . With a little work, the solution is found to be .2002 AMC 10B Answer Key1.E2. C3. A4. D5.E6. B7. E8. D9. D 10.C11.B 12. E 13. D 14. B 15. E 16.D 17.C 18.D 19.C 20.B21.B 22. B 23. D 24. D 25.A。
2019年美国数学竞赛(AMC10B)的试题与解答

这时第二个容器里充满了 3 的水. 问小容器与大容器的容积 4
之比是多少?
解: 设第一、二个容器的容积分别为 V1 和 V2, 依题意有
5 6 V1 =
3 4 V2,
从而
V1 V2
=
3 4 5 6
=
9 , 故 (D) 正确. 10
2. Consider the statement, ”If n is not prime, then n − 2 is
√ 由垂线段最短知, m2 + n2
|√am + bn|
=
√ 5.
当且仅当
a2 + b2
OA 与直线 ax + by = 5 垂直即 an = bm 时取最小值.
解法 2 由柯西不等式, (a2 +b2)(m2 +n2) (am+bn)2,
即 5(m2 + n2) 等号成立.
√ 25, m2 + n2
6 ter and the second was empty. She poured all the water from the
first container into the second container, at which point the sec3
ond container was full of water. What is the ratio of the volume 4
解: 反例是指符合命题的条件但不符合命题的结论的例
子, 即满足“若 n 不是素数, 则 n − 2 是素数. ”选项中只有 27
满足, 故 (E) 正确.
评注 运用柯西不等式关键是“配凑”形式, 充分利用已
知条件. 当出现分式和的形式时, 一般构造分式分母和作为
AMC 美国数学竞赛 2003 AMC 10B 试题及答案解析
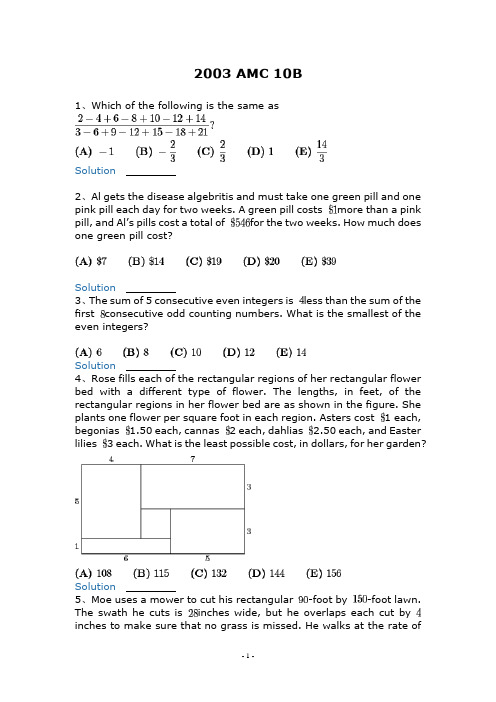
2003 AMC 10B1、Which of the following is the same asSolution2、Al gets the disease algebritis and must take one green pill and one pink pill each day for two weeks. A green pill costs more than a pink pill, and Al’s pills cost a total of for the two weeks. How much does one green pill cost?Solution3、The sum of 5 consecutive even integers is less than the sum of the first consecutive odd counting numbers. What is the smallest of the even integers?Solution4、Rose fills each of the rectangular regions of her rectangular flower bed with a different type of flower. The lengths, in feet, of the rectangular regions in her flower bed are as shown in the figure. She plants one flower per square foot in each region. Asters cost 1 each, begonias 1.50 each, cannas 2 each, dahlias 2.50 each, and Easter lilies 3 each. What is the least possible cost, in dollars, for her garden? Solution5、Moe uses a mower to cut his rectangular -foot by -foot lawn. The swath he cuts is inches wide, but he overlaps each cut by inches to make sure that no grass is missed. He walks at the rate offeet per hour while pushing the mower. Which of the following is closest to the number of hours it will take Moe to mow his lawn?Solution.6、Many television screens are rectangles that are measured by the length of their diagonals. The ratio of the horizontal length to the height in a standard television screen is . The horizontal length of a “-inch” television screen is closest, in inches, to which of the following?Solution7、The symbolism denotes the largest integer not exceeding . For example. , and . ComputeSolution.8、The second and fourth terms of a geometric sequence are and . Which of the following is a possible first term?Solution9、Find the value of that satisfies the equationSolution10、Nebraska, the home of the AMC, changed its license plate scheme. Each old license plate consisted of a letter followed by four digits. Each new license plate consists of three letters followed by three digits. By how many times is the number of possible license plates increased? Solution11、A line with slope intersects a line with slope at the point . What is the distance between the -intercepts of these two lines?Solution12、Al, Betty, and Clare split among them to be invested in different ways. Each begins with a different amount. At the end of one year they have a total of . Betty and Clare have both doubled their money, whereas Al has managed to lose . What was Al’s original portion?Solution.13、Let denote the sum of the digits of the positive integer . For example, and . For how many two-digit values of is ?Solution14、Given that , where both and are positive integers, find the smallest possible value for .Solution15、There are players in a singles tennis tournament. The tournament is single elimination, meaning that a player who loses a match is eliminated. In the first round, the strongest players are given a bye, and the remaining players are paired off to play. After each round, the remaining players play in the next round. The match continues until only one player remains unbeaten. The total number of matches played isSolution16、A restaurant offers three desserts, and exactly twice as many appetizers as main courses. A dinner consists of an appetizer, a main course, and a dessert. What is the least number of main courses that the restaurant should offer so that a customer could have a different dinner each night in the year ?Solution.17、An ice cream cone consists of a sphere of vanilla ice cream and a right circular cone that has the same diameter as the sphere. If the ice cream melts, it will exactly fill the cone. Assume that the melted ice cream occupies of the volume of the frozen ice cream. What is the ratio of the cone’s height to its radius?Solution18、What is the largest integer that is a divisor offor all positive even integers ?Solution19、Three semicircles of radius are constructed on diameter of a semicircle of radius . The centers of the small semicircles divide into four line segments of equal length, as shown. What is the area of the shaded region that lies within the large semicircle but outside the smaller semicircles?Solution20、In rectangle , and . Points and are onso that and . Lines and intersect at . Find the area of .Solution21、A bag contains two red beads and two green beads. You reach into the bag and pull out a bead, replacing it with a red bead regardless of the color you pulled out. What is the probability that all beads in the bag are red after three such replacements?Solution22、A clock chimes once at minutes past each hour and chimes on the hour according to the hour. For example, at 1 PM there is one chime and at noon and midnight there are twelve chimes. Starting at 11:15 AM on February , , on what date will the chime occur?Solution23、A regular octagon has an area of one square unit. What is the area of the rectangle ?Solution24、The first four terms in an arithmetic sequence are , , , and , in that order. What is the fifth term?Solution25、How many distinct four-digit numbers are divisible by and have as their last two digits?Solution。
amc10b 2023 题目pdf

vb6程序设计手册1. VB6基础知识与语法1.1 VB6概述与历史:1.1.1 VB6简介:Visual Basic 6.0(VB6)是一种基于事件驱动的编程语言,由微软开发,用于Windows应用程序的开发。
1.1.2 发展历史:VB6于1998年发布,成为当时Windows 平台上最受欢迎的应用程序开发工具之一。
1.2 VB6语法要点:1.2.1 事件驱动编程:VB6采用事件驱动的编程模型,程序通过对用户或系统事件的响应来执行相应的代码。
1.2.2 对象导向特性:VB6支持对象导向编程,允许开发者创建和操作各种对象,提高代码的模块化和可维护性。
1.3 VB6程序结构:1.3.1 模块与过程:VB6程序由模块组成,模块包含过程(Sub 和Function)以执行特定任务。
1.3.2 窗体与控件:窗体是VB6应用程序的可视化界面,控件用于与用户交互,例如按钮、文本框等。
1.4 数据类型与变量:1.4.1 基本数据类型:VB6包括整数、浮点数、字符串等基本数据类型,每种类型都有其特定的用途。
1.4.2 变量声明与作用域:学习如何声明变量以及它们的作用域是VB6编程的基础。
1.5 异常处理与调试:1.5.1 错误处理机制:VB6提供了异常处理机制,通过On Error 语句来处理运行时错误。
1.5.2 调试工具:学习使用VB6集成的调试工具,如断点、监视窗口等,提高程序调试效率。
2. VB6高级特性与设计模式2.1 ADO数据库编程:2.1.1 数据库连接与操作:学习如何使用VB6中的ActiveX Data Objects(ADO)来连接和操作数据库。
2.1.2 数据绑定:探讨在VB6中如何实现数据绑定,将数据库数据与用户界面关联起来。
2.2 多线程与异步编程:2.2.1 多线程概念:理解多线程编程的基本概念,以提高程序的并发性。
2.2.2 异步编程模式:学习在VB6中实现异步编程,以避免阻塞用户界面的情况。
amc 美国数学竞赛 amc 10b 试题及答案解析

2004 AMC 10BProblem 1Each row of the Misty Moon Amphitheater has 33 seats. Rows 12 through 22 are reserved for a youth club. How many seats are reserved for this club?There are rows of seats, giving seats.Problem 2How many two-digit positive integers have at least one 7 as a digit?Ten numbers () have as the tens digit. Nine numbers () have it as the ones digit. Number is in both sets.Thus the result is .Problem 3At each basketball practice last week, Jenny made twice as many free throws as she made at the previous practice. At her fifth practice she made48 free throws. How many free throws did she make at the first practice?At the fourth practice she made throws, at the third one it was , then we get throws for the second practice, and finally throws at the first one.Problem 4A standard six-sided die is rolled, and P is the product of the five numbers that are visible. What is the largest number that is certain to divide P?Solution 1The product of all six numbers is . The products of numbers that can be visible are , , ..., . The answer to this problem is their greatest common divisor -- which is , where is the least common multiple of . Clearly and the answer is .Solution 2Clearly, can not have a prime factor other than , and .We can not guarantee that the product will be divisible by , as the number can end on the bottom.We can guarantee that the product will be divisible by (one of and will always be visible), but not by .Finally, there are three even numbers, hence two of them are always visible and thus the product is divisible by . This is the most we can guarantee, as when the is on the bottom side, the two visible even numbers are and , and their product is not divisible by .Hence .Problem 5In the expression , the values of , , , and are , , , and , although not necessarily in that order. What is the maximum possible value of the result?If or , the expression evaluates to .If , the expression evaluates to .Case remains.In that case, we want to maximize where .Trying out the six possibilities we get that the best one is, where .Problem 6Which of the following numbers is a perfect square?Using the fact that , we can write:▪▪▪▪▪Clearly is a square, and as , , and are primes, none of the other four are squares.Problem 7On a trip from the United States to Canada, Isabella took U.S. dollars. At the border she exchanged them all, receiving Canadian dollars for every U.S. dollars. After spending Canadian dollars, she had Canadian dollars left. What is the sum of the digits of ?Solution 1Isabella had Canadian dollars. Setting up an equation we get, which solves to , and the sum of digits of isSolution 2Each time Isabelle exchanges U.S. dollars, she gets Canadian dollars and Canadian dollars extra. Isabelle received a total of Canadian dollars extra, therefore she exchanged U.S. dollars times. Thus .Problem 8Minneapolis-St. Paul International Airport is 8 miles southwest of downtown St. Paul and 10 miles southeast of downtown Minneapolis. Which of the following is closest to the number of miles between downtown St. Paul and downtown Minneapolis?The directions "southwest" and "southeast" are orthogonal. Thus the described situation is a right triangle with legs 8 miles and 10 mileslong. The hypotenuse length is , and thus the answer is .Without a calculator one can note that . Problem 9A square has sides of length 10, and a circle centered at one of its vertices has radius 10. What is the area of the union of the regions enclosed by the square and the circle?The area of the circle is , the area of the square is . Exactly of the circle lies inside the square. Thus the total area is.Problem 10A grocer makes a display of cans in which the top row has one can and each lower row has two more cans than the row above it. If the display contains cans, how many rows does it contain?The sum of the first odd numbers is . As in our case , we have .Problem 11Two eight-sided dice each have faces numbered 1 through 8. When the dice are rolled, each face has an equal probability of appearing on the top. What is the probability that the product of the two top numbers is greater than their sum?Solution 1We have , hence if at least one of the numbers is , the sum is larger. There such possibilities.We have .For we already have , hence all other cases are good.Out of the possible cases, we found that in the sum is greater than or equal to the product, hence in it is smaller. Therefore the answer is .Solution 2Let the two rolls be , and .From the restriction:Since and are non-negative integers between and , either , , orif and only if or .There are ordered pairs with , ordered pairs with , and ordered pair with and . So, there areordered pairs such that .if and only if and or equivalently and . This gives ordered pair .So, there are a total of ordered pairs with .Since there are a total of ordered pairs , there are ordered pairs with .Thus, the desired probability is .Problem 12An annulus is the region between two concentric circles. The concentric circles in the figure have radii and , with . Let be a radius of the larger circle, let be tangent to the smaller circle at , and let be the radius of the larger circle that contains . Let , , and . What is the area of the annulus?The area of the large circle is , the area of the small one is , hence the shaded area is .From the for the right triangle we have , hence and thus the shaded area is .Problem 13In the United States, coins have the following thicknesses: penny, mm; nickel, mm; dime, mm; quarter, mm. If a stack of these coins is exactly mm high, how many coins are in the stack?All numbers in this solution will be in hundreds of a millimeter.The thinnest coin is the dime, with thickness . A stack of dimes has height .The other three coin types have thicknesses , , and . By replacing some of the dimes in our stack by other, thicker coins, we can clearly create exactly all heights in the set.If we take an odd , then all the possible heights will be odd, and thus none of them will be . Hence is even.If the stack will be too low and if it will be too high. Thus we are left with cases and .If the possible stack heights are , with the remaining ones exceeding .Therefore there are coins in the stack.Using the above observation we can easily construct such a stack. A stack of dimes would have height , thus we need to add . This can be done for example by replacing five dimes by nickels (for ), and one dime by a penny (for ).Problem 14A bag initially contains red marbles and blue marbles only, with moreblue than red. Red marbles are added to the bag until only of the marbles in the bag are blue. Then yellow marbles are added to the baguntil only of the marbles in the bag are blue. Finally, the number of blue marbles in the bag is doubled. What fraction of the marbles now in the bag are blue?We can ignore most of the problem statement. The only important information is that immediately before the last step blue marbles formed of the marbles in the bag. This means that there were blue and other marbles, for some . When we double the number of blue marbles, there will be blue and other marbles, hence bluemarbles now form of all marbles in the bag.Problem 15Patty has coins consisting of nickels and dimes. If her nickels were dimes and her dimes were nickels, she would have cents more. How much are her coins worth?Solution 1She has nickels and dimes. Their total cost iscents. If the dimes were nickels and vice versa, she would havecents. This value should be cents more than the previous one. We get , which solves to . Her coins are worth .Solution 2Changing a nickel into a dime increases the sum by cents, and changing a dime into a nickel decreases it by the same amount. As the sum increased by cents, there are more nickels than dimes. As the total count is , this means that there are nickels and dimes.Problem 16Three circles of radius are externally tangent to each other and internally tangent to a larger circle. What is the radius of the large circle?The situation in shown in the picture below. The radius we seek is . Clearly . The point is clearly the center of the equilateral triangle , thus is of the altitude of this triangle. We get that . Therefore the radius we seek is.WARNING. Note that the answer does not correspond to any of the five options. Most probably there is a typo in option D.Problem 17The two digits in Jack's age are the same as the digits in Bill's age, but in reverse order. In five years Jack will be twice as old as Bill will be then. What is the difference in their current ages?Solution 1If Jack's current age is , then Bill's current age is .In five years, Jack's age will be and Bill's age will be .We are given that . Thus .For we get . For and the value is not an integer, and for it is more than . Thus the only solution is , and the difference in ages is .Solution 2Age difference does not change in time. Thus in five years Bill's age will be equal to their age difference.The age difference is , hence it is a multiple of . Thus Bill's current age modulo must be .Thus Bill's age is in the set .As Jack is older, we only need to consider the cases where the tens digit of Bill's age is smaller than the ones digit. This leaves us with the options .Checking each of them, we see that only works, and gives the solution .Problem 18In the right triangle , we have , , and . Points , , and are located on , , and , respectively, so that , , and . What is the ratio of the area of to that of ?First of all, note that , and therefore.Draw the height from onto as in the picture below:Now consider the area of . Clearly the triangles and are similar, as they have all angles equal. Their ratio is ,hence . Now the area of can be computed as= . Similarly we can find that as well.Hence , and the answer is .Problem 19In the sequence , , , , each term after the third is found by subtracting the previous term from the sum of the two terms that precede that term. For example, the fourth term is. What is the term in this sequence?Solution 1We already know that , , , and . Let's compute the next few terms to get the idea how the sequence behaves. We get ,, , and so on.We can now discover the following pattern: and . This is easily proved by induction. It follows that.Solution 2Note that the recurrence can be rewritten as.Hence we get that and alsoFrom the values given in the problem statement we see that .From we get that .From we get that .Following this pattern, we get.Problem 20In points and lie on and , respectively. If and intersect at so that and , what is ?Solution (Triangle Areas)We use the square bracket notation to denote area.Without loss of generality, we can assume . Then , and . We have , so we need to find the area of quadrilateral .Draw the line segment to form the two triangles and . Let , and . By considering trianglesand , we obtain , and by considering triangles and , we obtain . Solving, we get , , so the area of quadrilateral is .ThereforeSolution (Mass points)The presence of only ratios in the problem essentially cries out for mass points.As per the problem, we assign a mass of to point , and a mass of to . Then, to balance and on , has a mass of .Now, were we to assign a mass of to and a mass of to , we'd have . Scaling this down by (to get , which puts and in terms of the masses of and ), we assign a mass of to and a mass of to .Now, to balance and on , we must give a mass of . Finally, the ratio of to is given by the ratio of the mass of tothe mass of , which is .Solution (Coordinates)Affine transformations preserve ratios of distances, and for any pair of triangles there is an affine transformation that maps the first one onto the second one. This is why the answer is the same for any , and we just need to compute it for any single triangle.We can choose the points , , and . This way we will have , and . The situation is shown in the picture below:The point is the intersection of the lines and . The points on the first line have the form , the points on the second line have the form . Solving for we get , hence.The ratio can now be computed simply by observing the coordinates of , , and :Problem 21Let ; ; and ; ; be two arithmetic progressions. The set is the union of the first terms of each sequence. How many distinct numbers are in ?The two sets of terms are and.Now . We can compute. We will now find .Consider the numbers in . We want to find out how many of them lie in . In other words, we need to find out the number of valid values of for which .The fact "" can be rewritten as ", and ".The first condition gives , the second one gives .Thus the good values of are , and their count is .Therefore , and thus .Problem 22A triangle with sides of 5, 12, and 13 has both an inscribed and a circumscribed circle. What is the distance between the centers of those circles?This is obviously a right triangle. Pick a coordinate system so that the right angle is at and the other two vertices are at and .As this is a right triangle, the center of the circumcircle is in the middle of the hypotenuse, at .The radius of the inscribed circle can be computed using the well-known identity , where is the area of the triangle and its perimeter. In our case, and , thus . As the inscribed circle touches both legs, its center must be at .The distance of these two points is then.Problem 23Each face of a cube is painted either red or blue, each with probability 1/2. The color of each face is determined independently. What is the probability that the painted cube can be placed on a horizontal surface so that the four vertical faces are all the same color?Label the six sides of the cube by numbers to as on a classic dice. Then the "four vertical faces" can be: , , or .Let be the set of colorings where are all of the same color, similarly let and be the sets of good colorings for the other two sets of faces.There are possible colorings, and there are goodcolorings. Thus the result is . We need to compute .Using the we can writeClearly , as we have two possibilities for the common color of the four vertical faces, and two possibilities for each of the horizontal faces.What is ? The faces must have the same color, and at the same time faces must have the same color. It turns out thatthe set containing just the two cubes where all six faces have the same color.Therefore , and the result is .Problem 24In we have , , and . Point is on the circumscribed circle of the triangle so that bisects . What is the value of ?Problem 25A circle of radius is internally tangent to two circles of radius at points and , where is a diameter of the smaller circle. What is the area of the region, shaded in the picture, that is outside the smaller circle and inside each of the two larger circles?The area of the small circle is . We can add it to the shaded region, compute the area of the new region, and then subtract the area of the small circle from the result.Let and be the intersections of the two large circles. Connect them to and to get the picture below:Now obviously the triangles and are equilateral with side .Take a look at the bottom circle. The angle is , hence the sector is of the circle. The same is true for the sector of the bottom circle, and sectors and of the top circle.If we now sum the areas of these four sectors, we will almost get the area of the new shaded region - except that each of the two equilateral triangles will be counted twice.Hence the area of the new shaded region is, and the area of the original shared region is .。
2019年AMC10B美国数学竞赛(真题加详解)

2019 AMC 10B Problems/Problem 1The following problem is from both the 2019 AMC 10B #1 and 2019 AMC 12B #1, so both problems redirect to this page.ProblemAlicia had two containers. The first was full of water and the second was empty. She poured all the water from the first container into the secondcontainer, at which point the second container was full of water. What is the ratio of the volume of the first container to the volume of the secondcontainer?Solution 1Let the first jar's volume be and the second's be . It is giventhat . We find thatWe already know that this is the ratio of the smaller to the larger volumebecause it is less thanSolution 2We can set up a ratio to solve this problem. If is the volume of the firstcontainer, and is the volume of the second container, then:Cross-multiplying allows us to get . Thus the ratio of the volume of the first container to the second containeris .~IronicNinjaSolution 3An alternate solution is to plug in some maximum volume for the firstcontainer - let's say , so there was a volume of in the first container, and then the second container also has a volume of , so youget . Thus the answer is .2019 AMC 10B Problems/Problem 2The following problem is from both the 2019 AMC 10B #2 and 2019 AMC 12B #2, so both problems redirect to this page.ProblemConsider the statement, "If is not prime, then is prime." Which of the following values of is a counterexample to this statement?SolutionSince a counterexample must be value of which is not prime, must be composite, so we eliminate and . Now we subtract from the remaining answer choices, and we see that the only time is not prime iswhen .2019 AMC 10B Problems/Problem 3 ProblemIn a high school with students, of the seniors play a musical instrument, while of the non-seniors do not play a musical instrument. Inall, of the students do not play a musical instrument. How many non-seniors play a musical instrument?Solution 1of seniors do not play a musical instrument. If we denote as the numberof seniors, thenThus there are non-seniors. Since 70% of the non-seniorsplay a musical instrument, .~IronicNinjaSolution 2Let be the number of seniors, and be the number of non-seniors.ThenMultiplying both sides by gives usAlso, because there are 500 students in total.Solving these system of equations give us , .Since of the non-seniors play a musical instrument, the answer issimply of , which gives us .Solution 3 (using the answer choices)We can clearly deduce that of the non-seniors do play an instrument, but,since the total percentage of instrument players is , the non-senior population is quite low. By intuition, we can therefore see that the answer isaround or . Testing both of these gives us the answer . 2019 AMC 10B Problems/Problem 4 ProblemAll lines with equation such that form an arithmeticprogression pass through a common point. What are the coordinates of that point?Solution 1If all lines satisfy the condition, then we can just plug in values for , ,and that form an arithmetic progression. Let's use , , ,and , , . Then the two lines we get are:Use elimination to deduce and plug this into one of the previous line equations. We get Thus the common point is .~IronicNinjaSolution 2We know that , , and form an arithmetic progression, so if the commondifference is , we can say Now wehave , and expandinggives Factoringgives . Since this must always be true (regardless of the values of and ), we musthave and , so and the common point is .2019 AMC 10B Problems/Problem 5 ProblemTriangle lies in the first quadrant. Points , , and are reflected across the line to points , , and , respectively. Assume that none of the vertices of the triangle lie on the line . Which of the following statements is not always true?Triangle lies in the first quadrant.Triangles and have the same area.The slope of line is .The slopes of lines and are the same.Lines and are perpendicular to each other.SolutionLet's analyze all of the options separately.: Clearly is true, because a point in the first quadrant will have non-negative - and -coordinates, and so its reflection, with the coordinates swapped, will also have non-negative - and -coordinates.: The triangles have the same area,since and are the same triangle (congruent). More formally, we can say that area is invariant under reflection.: If point has coordinates , then will have coordinates .The gradient is thus , so this is true. (We know since the question states that none of the points , , or lies on the line , so there is no risk of division by zero).: Repeating the argument for , we see that both lines have slope , so this is also true.: By process of elimination, this must now be the answer. Indeed, ifpoint has coordinates and point has coordinates ,then and will, respectively, have coordinates and . The product of the gradientsof and is , so in fact these lines are never perpendicular to each other (using the "negative reciprocal" condition for perpendicularity).Thus the answer is .CounterexamplesIf and , then the slopeof , , is , while the slope of , ,is . is the reciprocal of , but it is not the negative reciprocal of . To generalize, let denote thecoordinates of point , let denote the coordinates of point ,let denote the slope of segment , and let denote the slope of segment . Then, the coordinates of are , andof are . Then, ,and .If and , , and in these cases, the condition is false.2019 AMC 10B Problems/Problem 6The following problem is from both the 2019 AMC 10B #6 and 2019 AMC 12B #4, so both problems redirect to this page.ProblemThere is a real such that .What is the sum of the digits of ?Solution 1Solving by the quadraticformula,(since clearly ). The answer is therefore .~IronicNinjaSolution 2Dividing both sidesby givesSince is non-negative, . The answer is .Solution 3Dividing both sides by as beforegives . Now factorout , giving . By considering the prime factorization of , a bit of experimentation givesus and , so , so the answeris .2019 AMC 10B Problems/Problem 7The following problem is from both the 2019 AMC 10B #7 and 2019 AMC 12B #5, so both problems redirect to this page.ProblemEach piece of candy in a store costs a whole number of cents. Casper has exactly enough money to buy either pieces of red candy, pieces of green candy, pieces of blue candy, or pieces of purple candy. A piece of purple candy costs cents. What is the smallest possible value of ?Solution 1If he has enough money to buy pieces of red candy, pieces of green candy, and pieces of blue candy, then the smallest amount of money hecould have is cents. Since a piece of purple candy costs cents, the smallest possible valueof is .~IronicNinjaSolution 2We simply need to find a value of that is divisible by , , and .Observe that is divisible by and , but not . is divisible by , , and , meaning that we have exact change (in this case, cents) to buy each type of candy, so the minimum valueof is .2019 AMC 10B Problems/Problem 8ProblemThe figure below shows a square and four equilateral triangles, with each triangle having a side lying on a side of the square, such that each triangle has side length and the third vertices of the triangles meet at the center of the square. The region inside the square but outside the triangles is shaded. What is the area of the shaded region?Solution 1We notice that the square can be split into congruent smaller squares, with the altitude of the equilateral triangle being the side of this smaller square. Therefore, the area of each shaded part that resides within a square is the total area of the square subtracted from each triangle (which has already been split in half). When we split an equilateral triangle in half, we gettwo triangles. Therefore, the altitude, which is also the side length of one of the smaller squares, is . We can then compute the areaof the two triangles as .The area of the each small squares is the square of the side length,i.e. . Therefore, the area of the shaded region in each of the four squares is .Since there are of these squares, we multiply this by toget as our answer.Solution 2We can see that the side length of the square is by considering thealtitude of the equilateral triangle as in Solution 1. Using the Pythagorean Theorem, the diagonal of the square isthus . Because of this, the height of one ofthe four shaded kites is . Now, we just need to find the length of that kite. By the Pythagorean Theorem again, this lengthis . Nowusing , the area of one of the four kitesis . 2019 AMC 10B Problems/Problem 9 ProblemThe function is defined by for all real numbers , where denotes the greatest integer less than or equal to the real number . What is the range of ?Solution 1There are four cases we need to consider here.Case 1: is a positive integer. Without loss of generality, assume . Then .Case 2: is a positive fraction. Without loss of generality, assume .Then .Case 3: is a negative integer. Without loss of generality, assume . Then .Case 4: is a negative fraction. Without loss of generality, assume . Then .Thus the range of the function is .~IronicNinja, edited by someone elseSolution 2It is easily verified that when is an integer, is zero. We therefore need only to consider the case when is not an integer.When is positive, , soWhen is negative, let be composed of integer part and fractional part (both ):Thus, the range of f is .Note: One could solve the case of as a negative non-integer in thisway:2019 AMC 10B Problems/Problem 10The following problem is from both the 2019 AMC 10B #10 and 2019 AMC 12B #6, so both problems redirect to this page.ProblemIn a given plane, points and are units apart. How manypoints are there in the plane such that the perimeterof is units and the area of is square units?Solution 1Notice that whatever point we pick for , will be the base of thetriangle. Without loss of generality, letpoints and be and , since for any other combination of points, we can just rotate the plane to makethem and under a new coordinate system. When we pickpoint , we have to make sure that its -coordinate is , because that's the only way the area of the triangle can be .Now when the perimeter is minimized, by symmetry, we put in the middle, at . We can easily see that and will bothbe . The perimeter of this minimal triangleis , which is larger than . Since the minimum perimeter is greater than , there is no triangle that satisfies the condition, givingus .~IronicNinjaSolution 2Without loss of generality, let be a horizontal segment of length .Now realize that has to lie on one of the lines parallel to andvertically units away from it. But is already 50, andthis doesn't form a triangle. Otherwise, without loss ofgenerality, . Dropping altitude , we have a righttriangle with hypotenuse and leg , which is clearly impossible, again giving the answer as .2019 AMC 10B Problems/Problem 11 ProblemTwo jars each contain the same number of marbles, and every marble is either blue or green. In Jar the ratio of blue to green marbles is , and the ratio of blue to green marbles in Jar is . There are green marbles in all. How many more blue marbles are in Jar than in Jar ?SolutionCall the number of marbles in each jar (because the problem specifies that they each contain the same number). Thus, is the number of green marbles in Jar , and is the number of green marbles in Jar .Since , we have , so thereare marbles in each jar.Because is the number of blue marbles in Jar , and is the number of blue marbles in Jar , there are more marbles in Jar than Jar . This means the answer is .2019 AMC 10B Problems/Problem 12 ProblemWhat is the greatest possible sum of the digits in the base-seven representation of a positive integer less than ?Solution 1Observe that . To maximize the sum of the digits, we want as many s as possible (since is the highest value in base ), and this will occur with either of the numbers or . Thus, the answeris .~IronicNinja, edited by some peopleNote: the number can also be , which will also give the answer of . Solution 2Note that all base numbers with or more digits are in fact greaterthan . Since the first answer that is possible using a digit number is , we start with the smallest base number that whose digits sum to ,namely . But this is greater than , so we continue bytrying , which is less than 2019. So the answer is .2019 AMC 10B Problems/Problem 13The following problem is from both the 2019 AMC 10B #13 and 2019 AMC 12B #7, so both problems redirect to this page.ProblemWhat is the sum of all real numbers for which the median of thenumbers and is equal to the mean of those five numbers?SolutionThe mean is .There are three possibilities for the median: it is either , , or .Let's start with .has solution , and the sequenceis , which does have median , so this is a valid solution.Now let the median be .gives , so the sequence is , which has median , so this is not valid.Finally we let the median be ., and the sequence is , which has median . This case is therefore again not valid.Hence the only possible value of is2019 AMC 10B Problems/Problem 14 ProblemThe base-ten representationfor is , where , ,and denote digits that are not given. What is ?Solution 1We can figure out by noticing that will end with zeroes, as there are three s in its prime factorization. Next, we use the fact that is a multiple of both and . Their divisibility rules (see Solution 2) tell usthat and that . By inspection, we see that is a valid solution. Therefore the answer is .Solution 2 (similar to Solution 1)We know that and are both factors of . Furthermore, we knowthat , because ends in three zeroes (see Solution 1). We can simply use the divisibility rules for and for this problem to find and . For to be divisible by , the sum of digits must simply be divisible by .Summing the digits, we get that must be divisible by . Thisleaves either or as our answer choice. Now we test for divisibility by . For a number to be divisible by , the alternating sum must be divisibleby (for example, with the number , ,so is divisible by ). Applying the alternating sum test to this problem, we see that must be divisible by 11. By inspection, we can see that this holds if and . The sumis .2019 AMC 10B Problems/Problem 15ProblemRight triangles and , have areas of 1 and 2, respectively. A side of iscongruent to a side of , and a different side of is congruent to a differentside of . What is the square of the product of the lengths of the other (third)side of and ?Solution 1First of all, let the two sides which are congruent be and , where . The only way that the conditions of the problem can be satisfied is if is the shorter leg of and the longer leg of , and is the longer leg of and thehypotenuse of .Notice that this means the value we are looking for is the squareof , which is just . The area conditions give us twoequations: and .This means that and that .Taking the second equation, we get , sosince , .Since , we get .The value we are looking for is just so the answer is .Solution bySolution 2Like in Solution 1, we have and .Squaring both equations yields and .Let and . Then ,and , so .We are looking for the value of , so the answeris .Solution 3Firstly, let the right triangles be and ,with being the smaller triangle. As in Solution 1,let and . Additionally,let and .We are given that and , sousing , we have and . Dividing the twoequations, we get = , so .Thus is a right triangle, meaningthat . Now by the Pythagorean Theoremin ,.The problem requires the square of the product of the third side lengths of each triangle, which is . By substitution, we seethat = . We alsoknow.Since we want , multiplying both sides by getsus . Now squaringgives .2019 AMC 10B Problems/Problem 16ProblemIn with a right angle at , point lies in the interior of andpoint lies in the interior of so that and the ratio . What is the ratioSolution 1Without loss of generality, let and . Let and .As and areisosceles, and .Then , so isa triangle with .Then , and is a triangle.In isosceles triangles and , drop altitudesfrom and onto ; denote the feet of these altitudesby and respectively. Then by AAA similarity, so we get that ,and . Similarly we get ,and .Solution 2Let , and . (For thissolution, is above , and is to the right of ). Also let ,so , whichimplies . Similarly, , whichimplies . This further impliesthat .Now we seethat. Thus is a right triangle, with side lengths of , , and (by the Pythagorean Theorem, or simply the Pythagorean triple ).Therefore (by definition), ,and . Hence (by thedouble angle formula), giving .By the Law of Cosines in , if , wehaveNow . Thus theanswer is .~IronicNinjaSolution 3Draw a nice big diagram and measure. The answers to this problem are not very close, so it is quite easy to get to the correct answer by simply drawing a diagram. (Note: this strategy should only be used as a last resort!)2019 AMC 12B Problems/Problem 13(Redirected from 2019 AMC 10B Problems/Problem 17)The following problem is from both the 2019 AMC 10B #17 and 2019 AMC 12B #13, so both problems redirect to this page.ProblemA red ball and a green ball are randomly and independently tossed into binsnumbered with the positive integers so that for each ball, the probability that it is tossed into bin is for What is the probability that the red ball is tossed into a higher-numbered bin than the green ball?Solution 1By symmetry, the probability of the red ball landing in a higher-numbered bin is the same as the probability of the green ball landing in a higher-numbered bin. Clearly, the probability of both landing in the same binis (by the geometric series sum formula). Therefore the other two probabilities have to bothbe .Solution 2Suppose the green ball goes in bin , for some . The probability of this occurring is . Given that this occurs, the probability that the red ball goesin a higher-numbered bin is (by thegeometric series sum formula). Thus the probability that the green ball goesin bin , and the red ball goes in a bin greater than , is . Summing from to infinity, we getwhere we again used the geometric series sum formula. (Alternatively, if this sum equals , then by writing out the terms andmultiplying both sides by , we see , which gives .) Solution 3The probability that the two balls will go into adjacent binsisby the geometric series sum formula. Similarly, the probability that the two balls will go into bins that have a distance of from each otheris(again recognizing a geometric series). We can see that each time we add a bin between the two balls, the probability halves. Thus, our answeris , which, by the geometric series sum formula,is .-fidgetboss_4000Solution 4 (quick, conceptual)Define a win as a ball appearing in higher numbered box.Start from the first box.There are possible results in the box: Red, Green, Red and Green, ornone, with an equal probability of for each. If none of the balls is in the first box, the game restarts at the second box with the same kind of probability distribution, so if is the probability that Red wins, we canwrite : there is a probability that "Red" wins immediately,a probability in the cases "Green" or "Red and Green", and in the "None"case (which occurs with probability), we then start again, giving the sameprobability . Hence, solving the equation, we get . Solution 5Write out the infinite geometric series as , . To find the probablilty that red goes in a higher-numbered bin than green, we can simply remove all odd-index terms (i.e term , term , etc.), and then sum theremaining terms - this is in fact precisely equivalent to the method of Solution2. Writing this out as another infinite geometric sequence, we are leftwith . Summing, we get2019 AMC 10B Problems/Problem 18 ProblemHenry decides one morning to do a workout, and he walks of the way from his home to his gym. The gym is kilometers away from Henry's home. At thatpoint, he changes his mind and walks of the way from where he is back toward home. When he reaches that point, he changes his mind again and walks ofthe distance from there back toward the gym. If Henry keeps changing his mindwhen he has walked of the distance toward either the gym or home from the point where he last changed his mind, he will get very close to walking back and forth between a point kilometers from home and a point kilometers fromhome. What is ?Solution 1Let the two points that Henry walks in between be and , with being closer to home. As given in the problem statement, the distances of thepoints and from his home are and respectively. By symmetry, the distance of point from the gym is the same as the distance from home to point . Thus, . In addition, when he walks from point to home, he walks of the distance, ending at point . Therefore, we knowthat . By substituting, we get .Adding these equations now gives . Multiplying by , we get ,so .Solution 2 (not rigorous)We assume that Henry is walking back and forth exactly betweenpoints and , with closer to Henry's home than . Denote Henry's home as a point and the gym as a point .Then and ,so .Therefore,. 2019 AMC 10B Problems/Problem 19The following problem is from both the 2019 AMC 10B #19 and 2019 AMC 12B #14, so both problems redirect to this page.ProblemLet be the set of all positive integer divisors of How many numbers are the product of two distinct elements ofSolutionThe prime factorization of is . Thus, we choose twonumbers and where and, whose product is ,where and .Notice that this is analogous to choosing a divisorof , whichhas divisors. However, some of thedivisors of cannot be written as a product of two distinct divisorsof , namely: , , , and . The last twocannot be so written because the maximum factor of containingonly s or s (and not both) is only or . Since the factors chosen must be distinct, the last two numbers cannot be so written because they would require or . This gives candidatenumbers. It is not too hard to show that every number of the form ,where , and are not both or , can be written asa product of two distinct elements in . Hence the answer is . 2019 AMC 10B Problems/Problem 20The following problem is from both the 2019 AMC 10B #20 and 2019 AMC 12B #15, so both problems redirect to this page.ProblemAs shown in the figure, line segment is trisected bypoints and so that Three semicirclesof radius and have their diameters on and are tangent to line at and respectively. A circle ofradius has its center on The area of the region inside the circle butoutside the three semicircles, shaded in the figure, can be expressed in the form where and are positive integersand and are relatively prime. What is ?SolutionDivide the circle into four parts: the top semicircle (); the bottom sector (), whose arc angle is because the large circle's radius is and the short length (the radius of the smaller semicircles) is , givinga triangle; the triangle formed by the radii of and the chord (), and the four parts which are the corners of a circle inscribedin a square (). Then the area is (in , wefind the area of the shaded region above the semicircles but below the diameter, and in we find the area of the bottom shaded region).The area of is .The area of is .For the area of , the radius of , and the distance of (the smaller semicircles' radius) to , creates two triangles,so 's area is .The area of is .Hence, finding , the desired areais , so the answeris2019 AMC 10B Problems/Problem 21 ProblemDebra flips a fair coin repeatedly, keeping track of how many heads and how many tails she has seen in total, until she gets either two heads in a row or two tails in a row, at which point she stops flipping. What is the probability that she gets two heads in a row but she sees a second tail before she sees a second head?SolutionWe firstly want to find out which sequences of coin flips satisfy the given condition. For Debra to see the second tail before the seecond head, her first flip can't be heads, as that would mean she would either end with double tails before seeing the second head, or would see two heads before she sees two tails. Therefore, her first flip must be tails. The shortest sequence of flips by which she can get two heads in a row and see the second tail before she sees the secondhead is , which has a probability of . Furthermore, she can prolong her coin flipping by adding an extra , which itself has aprobability of . Since she can do this indefinitely, this gives an infinite geometric series, which means the answer (by the geometric series sum formula)is .Solution 2 (Easier)Note that the sequence must start in THT, which happens with probability. Now, let be the probability that Debra will get two heads in a row after flippingTHT. Either Debra flips two heads in a row immediately (probability ), or flips a head and then a tail and reverts back to the "original position" (probability ). Therefore, , so , so our final answeris . -Stormersyle get rect2019 AMC 10B Problems/Problem 22The following problem is from both the 2019 AMC 10B #22 and 2019 AMC 12B #19, so both problems redirect to this page.ProblemRaashan, Sylvia, and Ted play the following game. Each starts with . A bell rings every seconds, at which time each of the players who currently have money simultaneously chooses one of the other two playersindependently and at random and gives to that player. What is theprobability that after the bell has rung times, each player willhave ? (For example, Raashan and Ted may each decide to give to Sylvia, and Sylvia may decide to give her her dollar to Ted, at which pointRaashan will have , Sylvia will have , and Ted will have , and that is the end of the first round of play. In the second round Rashaan has no money to give, but Sylvia and Ted might choose each other to give their to, and the holdings will be the same at the end of the second round.)SolutionOn the first turn, each player starts off with . Each turn after that, there are only two possibilities: either everyone stays at , which we will writeas , or the distribution of moneybecomes in some order, which we writeas . We will consider these two states separately.In the state, each person has two choices for whom to give their dollar to, meaning there are possible ways that the money canbe rearranged. Note that there are only two ways that we canreach again:1. Raashan gives his money to Sylvia, who gives her money to Ted, who gives his money to Raashan.2. Raashan gives his money to Ted, who gives his money to Sylvia, who gives her money to Raashan.Thus, the probability of staying in the state is , while theprobability of going to the state is (we can check that the 6 other possibilities lead to )In the state, we will label the person with as person A, the person with as person B, and the person with as person C.Person A has two options for whom to give money to, and person B has 2 options for whom to give money to, meaning there are。
AMC 10B 试题及答案解析

2010 AMC 10B1 . What is ?SolutionWe first expand the first term, simplify, and then compute to get an answer of .2 、Makarla attended two meetings during her -hour work day. The first meeting took minutes and the second meeting took twice as long. What percent of her work day was spent attending meetings?SolutionThe percentage of her time spent in meetings is the total amount of time spent in meetings divided by the length of her workday.The total time spent in meetings isTherefore, the percentage is3、A drawer contains red, green, blue, and white socks with at least 2 of each color. What is the minimum number of socks that must be pulled from the drawer to guarantee a matching pair?SolutionAfter you draw socks, you can have one of each color, so (according to the pigeonhole principle), if you pull then you will be guaranteed a matching pair.4 、For a real number , define to be the average of and . What is ?SolutionThe average of two numbers, and , is defined as . Thus the average of and would be . With that said, we need to find the sum when we plug, , and into that equation. So:.5 、A month with days has the same number of Mondays and Wednesdays.How many of the seven days of the week could be the first day of this month?Solution(B). 36 、A circle is centered at , is a diameter and is a point on the circle with . What is the degree measure of ?SolutionAssuming the reader is not readily capable to understand how will always be right, the I will continue with an easily understandable solution. Since is the center, are all radii, they are congruent. Thus, and are isosceles triangles. Also, note thatand are supplementary, then . Since is isosceles, then . They also sum to , so each angle is .7 、A triangle has side lengths , , and . A rectangle has width and area equal to the area of the rectangle. What is the perimeter of this rectangle?SolutionThe triangle is isosceles. The height of the triangle is therefore given byNow, the area of the triangle isWe have that the area of the rectangle is the same as the area of the triangle, namely 48. We also have the width of the rectangle: 4.The length of the rectangle therefor is:The perimeter of the rectangle then becomes:The answer is:8 、A ticket to a school play cost dollars, where is a whole number. A group of 9th graders buys tickets costing a total of $, and a group of 10th graders buys tickets costing a total of $. How many values for are possible?SolutionWe see how many common integer factors 48 and 64 share. Of the factors of 48 - 1, 2, 3, 4, 6, 8, 12, 16, 24, and 48; only 1, 2, 4, 8, and 16 are factors of 64. So there are possibilities for the ticket price.9 、Lucky Larry's teacher asked him to substitute numbers for , , , , and in the expression and evaluate the result. Larry ignored the parenthese but added and subtracted correctly andobtained the correct result by coincidence. The number Larry sustitued for , , , and were , , , and , respectively. What number did Larry substitude for ?SolutionSimplify the expression . I recommend to start with the innermost parenthesis and work your way out.So you get:Henry substituted with respectively.We have to find the value of , such that(the same expression without parenthesis).Substituting and simplifying we get:So Henry must have used the value for .Our answer is:10、Shelby drives her scooter at a speed of miles per hour if it is not raining, and miles per hour if it is raining. Today she drove in the sun in the morning and in the rain in the evening, for a total of miles in minutes. How many minutes did she drive in the rain?SolutionWe know thatSince we know that she drove both when it was raining and when it was not and that her total distance traveled is miles.We also know that she drove a total of minutes which is of an hour. We get the following system of equations, where is the time traveled when it was not raining and is the time traveled when it was raining:Solving the above equations by multiplying the second equation by 30 and subtracting the second equation from the first we get:We know now that the time traveled in rain was of an hour, which is minutesSo, our answer is:11 、A shopper plans to purchase an item that has a listed price greater than $and can use any one of the three coupns. Coupon A givesoff the listed price, Coupon B gives $off the listed price, and Coupon C gives off the amount by which the listed price exceeds $.Let and be the smallest and largest prices, respectively, for which Coupon A saves at least as many dollars as Coupon B or C. What is −?SolutionLet the listed price be , whereCoupon A saves us:Coupon B saves us:Coupon C saves us:Now, the condition is that A has to be greater than or equal to either B or C which give us the following inequalities:We see here that the greatest possible value for p is and the smallest isThe difference between and isOur answer is:12 、At the beginning of the school year, of all students in Mr. Wells' math class answered "Yes" to the question "Do you love math", andanswered "No." At the end of the school year, answered "Yes" and answerws "No." Altogether, of the students gave a differentanswer at the beginning and end of the school year. What is the difference between the maximum and the minimum possible values of ?SolutionThe minimum possible value occurs when of the students who originally answered "No." answer "Yes." In this case,The maximum possible value occurs when of the students who originally answered "Yes." answer "No." and the of the students who originally answered "No." answer "Yes." In this case,Subtract to obtain an answer of13 、What is the sum of all the solutions of ?SolutionCase 1:Case 1a:Case 1b:Case 2:Case 2a:Case 2b:Since an absolute value cannot be negative, we exclude . The answer is14 、The average of the numbers and is . What is ?SolutionWe must find the average of the numbers from to and in terms of . The sum of all these terms is . We must divide this by the total number of terms, which is . We get: . This is equal to , as stated in the problem. We have: . We can now cross multiply. This gives:This gives us our answer.15 、On a -question multiple choice math contest, students receive points for a correct answer, points for an answer left blank, and point for an incorrect answer. Jesse’s total score on the contest was . What is the maximum number of questions that Jesse could have answered correctly?SolutionLet be the amount of questions Jesse answered correctly, be the amount of questions Jesse left blank, and be the amount of questions Jesse answered incorrectly. Since there were questions on the contest, . Since his total score was , . Also,. We can substitute this inequality into the previous equation to obtain another inequality:. Since is an integer, the maximum value for is .16 、A square of side length and a circle of radius share the same center. What is the area inside the circle, but outside the square?Solution(B)17 、Every high school in the city of Euclid sent a team of students to a math contest. Each participant in the contest received a different score. Andrea's score was the median among all students, and hers was the highest score on her team. Andrea's teammates Beth and Carla placed th and th, respectively. How many schools are in the city?SolutionLet the be the number of schools, be the number of contestants, and be Andrea's score. Since the number of participants divided by three isthe number of schools, . Andrea received a higher score than her teammates, so . Since is the maximum possible median, then is the maximum possible number ofparticipants. Therefore, . This yields thecompound inequality: . Since a set with an even number of elements has a median that is the average of the two middle terms, an occurrence that cannot happen in this situation, cannot be even.is the only other option.18 、Positive integers , , and are randomly and independently selected with replacement from the set . What is the probability that is divisible by ?Solution(E)13/2719 、A circle with center has area . Triangle is equilateral,is a chord on the circle, , and point is outside . What is the side length of ?Solution(B)620 、Two circles lie outside regular hexagon . The first is tangent to , and the second is tangent to . Both are tangent to lines and . What is the ratio of the area of the second circle to that of the first circle?SolutionA good diagram here is very helpful.The first circle is in red, the second in blue. With this diagram, we can see that the first circle is inscribed in equilateral triangle while the second circle is inscribed in . From this, it's evident that the ratio of the red area to the blue area is equal to the ratio of the areas of triangles toSince the ratio of areas is equal to the square of the ratio of lengths, weknow our final answer is From the diagram, we can see that this isThe letter answer is D21 、A palindrome between and is chosen at random. What is the probability that it is divisible by ?SolutionView the palindrome as some number with form (decimal representation): . But because the number is a palindrome, . Recombining this yields . 1001 is divisible by 7, which means that as long as , the palindrome will be divisible by 7. This yields 9 palindromes out of 90 () possibilities for palindromes. However, if , then this givesanother case in which the palindrome is divisible by 7. This adds another 9 palindromes to the list, bringing our total to22 、Seven distinct pieces of candy are to be distributed among three bags. The red bag and the blue bag must each receive at least one piece of candy; the white bag may remain empty. How many arrangements are possible?SolutionWe can count the total number of ways to distribute the candies (ignoring the restrictions), and then subtract the overcount to get the answer.Each candy has three choices; it can go in any of the three bags.Since there are seven candies, that makes the total distributionsTo find the overcount, we calculate the number of invalid distributions: the red or blue bag is empty.The number of distributions such that the red bag is empty is equal to , since it's equivalent to distributing the 7 candies into 2 bags.We know that the number of distributions with the blue bag is empty will be the same number because of the symmetry, so it's also .The case where both the red and the blue bags are empty (all 7 candies are in the white bag) are included in both of the above calculations, and this case has only distribution.The total overcount isThe final answer will beThat makes the letter choice C23 、The entries in a array include all the digits from through ,arranged so that the entries in every row and column are in increasing order. How many such arrays are there?Solution(D)6024 、A high school basketball game between the Raiders and Wildcatswas tied at the end of the first quarter. The number of points scored by the Raiders in each of the four quarters formed an increasing geometric sequence, and the number of points scored by the Wildcats in each of the four quarters formed an increasing arithmetic sequence. At the end of the fourth quarter, the Raiders had won by one point. Neither team scored more than points. What was the total number of points scored by the two teams in the first half?SolutionRepresent the teams' scores as: andWe have Manipulating this, we can get, orSince both are increasing sequences, . We can check cases up to because when , we get . When▪▪▪Checking each of these cases individually back into the equation, we see that only when a=5 and n=2, we get an integer value for m, which is 9. The original question asks for the first half scores summed, so we must find25 、Let , and let be a polynomial with integer coefficients such that, and.What is the smallest possible value of ?SolutionThere must be some polynomial such thatThen, plugging in values of we getThus, the least value of must be the . Solving, we receive , so our answer is .。
-年amc 10a和b竞赛真题及答案(英文版)

2010-2015年AMC 10A和B竞赛真题及答案(英文版)
你好,目前,只分享了2010-2013年AMC 10A和B竞赛真题及答案(英文版),2014-2015年的暂时还没有上传,等采纳后再私信我吧!需要的话,我可以将Word文档中的原图文件一同发给你,求采纳,毕竟我花了差不多一个下午才整理完,谢谢!(@_@)
2010年AMC 10Aห้องสมุดไป่ตู้赛真题及答案(英文版)
2010年AMC 10B竞赛真题及答案(英文版)
2011年AMC 10A竞赛真题及答案(英文版)
2011年AMC 10B竞赛真题及答案(英文版)
Problem 4
2012年AMC 10A竞赛真题及答案(英文版)
go去wentgone
2012年AMC 10B竞赛真题及答案(英文版)
get得到gotgot
sink下沉sank / sunksunk / sunken
give给gavegiven
hit打hithit
不规则动词表
2013年AMC 10A竞赛真题及答案(英文版)
mistake误认mistookmistaken
grow成长grewgrown
think思考thoughtthought
2020 AMC 10B (美国数学竞赛)真题加详解

2020 AMC 10B Solution Problem1What is the value ofSolutionWe know that when we subtract negative numbers, .The equation becomesProblem2Carl has cubes each having side length , and Kate has cubes each having side length . What is the total volume of these cubes?SolutionA cube with side length has volume , so of these will have a total volume of .A cube with side length has volume , so of these will have a total volume of .~quacker88Problem 3The ratio of to is , the ratio of to is , and the ratioof to is . What is the ratio of toSolution 1WLOG, let and .Since the ratio of to is , we can substitute in the value of toget .The ratio of to is , so .The ratio of to is then so our answeris ~quacker88Solution 2We need to somehow link all three of the ratios together. We can start by connecting the last two ratios together by multiplying the last ratio by two., and since , we can link themtogether to get .Finally, since , we can link this again to get: ,so ~quacker88Problem4The acute angles of a right triangle are and , where andboth and are prime numbers. What is the least possible value of ?SolutionSince the three angles of a triangle add up to and one of the anglesis because it's a right triangle, .The greatest prime number less than is . If ,then , which is not prime.The next greatest prime number less than is . If ,then , which IS prime, so we have our answer ~quacker88 Solution 2Looking at the answer choices, only and are coprime to . Testing , the smaller angle, makes the other angle which is prime, therefore our answerisProblem5How many distinguishable arrangements are there of 1 brown tile, 1 purple tile, 2 green tiles, and 3 yellow tiles in a row from left to right? (Tiles of the same color are indistinguishable.)SolutionLet's first find how many possibilities there would be if they were all distinguishable, then divide out the ones we overcounted.There are ways to order objects. However, since there's ways to switch the yellow tiles around without changing anything (since they're indistinguishable) and ways to order the green tiles, we have to divide out these possibilities.~quacker88SolutionWe can repeat chooses extensively to find the answer. Thereare choose ways to arrange the brown tiles which is . Then from the remaining tiles there are choose ways to arrange the red tiles. And now from the remaining two tiles and two slots we can see there are two ways to arrange the purple and brown tiles, giving us an answerofProblem6Driving along a highway, Megan noticed that her odometershowed (miles). This number is a palindrome-it reads the same forward and backward. Then hours later, the odometer displayed the next higher palindrome. What was her average speed, in miles per hour, during this -hour period?SolutionIn order to get the smallest palindrome greater than , we need to raise the middle digit. If we were to raise any of the digits after the middle, we would be forced to also raise a digit before the middle to keep it a palindrome, making it unnecessarily larger.So we raise to the next largest value, , but obviously, that's not how place value works, so we're in the s now. To keep this a palindrome, our number is now .So Megan drove miles. Since this happened over hours, she drove at mph. ~quacker88 Problem7How many positive even multiples of less than are perfect squares?SolutionAny even multiple of is a multiple of , so we need to find multiples of that are perfect squares and less than . Any solution that we want will be in theform , where is a positive integer. The smallest possible value isat , and the largest is at (where the expression equals ). Therefore, there are a total of possible numbers.-PCChess Problem8Points and lie in a plane with . How many locations forpoint in this plane are there such that the triangle with vertices , ,and is a right triangle with area square units?Solution 1There are options here:1. is the right angle.It's clear that there are points that fit this, one that's directly to the rightof and one that's directly to the left. We don't need to find the length, we just need to know that it is possible, which it is.2. is the right angle.Using the exact same reasoning, there are also solutions for this one.3. The new point is the right angle.(Diagram temporarily removed due to asymptote error)The diagram looks something like this. We know that the altitude tobase must be since the area is . From here, we must see if there are valid triangles that satisfy the necessary requirements.First of all, because of the area.Next, from the Pythagorean Theorem.From here, we must look to see if there are valid solutions. There are multiple ways to do this:We know that the minimum value of iswhen . In this case, the equationbecomes , which is LESSthan . . The equationbecomes , which is obviously greater than . We canconclude that there are values for and in between that satisfy the Pythagorean Theorem.And since , the triangle is not isoceles, meaning we could reflectit over and/or the line perpendicular to for a total of triangles this case.Solution 2Note that line segment can either be the shorter leg, longer leg or thehypotenuse. If it is the shorter leg, there are two possible points for that cansatisfy the requirements - that being above or below . As such, thereare ways for this case. Similarly, one can find that there are also ways for point to lie if is the longer leg. If it is a hypotenuse, then thereare possible points because the arrangement of the shorter and longer legs can be switched, and can be either above or below the line segment. Therefore, the answer is .Problem9How many ordered pairs of integers satisfy theequationSolutionRearranging the terms and and completing the square for yields theresult . Then, notice that can onlybe , and because any value of that is greater than 1 will causethe term to be less than , which is impossible as must be real. Therefore, plugging in the above values for gives the orderedpairs , , , and gives a totalof ordered pairs.Solution 2Bringing all of the terms to the LHS, we see a quadraticequation in terms of . Applying the quadratic formula, weget In order for to be real, which it must be given the stipulation that we are seekingintegral answers, we know that the discriminant, must benonnegative. Therefore, Here, we see that we must split the inequality into a compound, resultingin .The only integers that satisfy this are . Plugging thesevalues back into the quadratic equation, we see that both produce a discriminant of , meaning that there is only 1 solution for .If , then the discriminant is nonzero, therefore resulting in two solutions for .Thus, the answer is .~TiblisSolution 3, x firstSet it up as a quadratic in terms of y:Then the discriminant is This will clearly only yield real solutionswhen , because it is always positive. Then . Checking each one: and are the same when raised to the 2020th power:This has only has solutions , so are solutions. Next, if :Which has 2 solutions, so andThese are the only 4 solutions, soSolution 4, y firstMove the term to the other side toget . Because for all , then . If or , the right side is and therefore . When , the right side become , therefore . Our solutions are , , , . There are solutions, so the answer is - wwt7535Problem 10A three-quarter sector of a circle of radius inches together with its interior can be rolled up to form the lateral surface area of a right circular cone by taping together along the two radii shown. What is the volume of the cone in cubicinches?SolutionNotice that when the cone is created, the radius of the circle will become the slant height of the cone and the intact circumference of the circle will become the circumference of the base of the cone.We can calculate that the intact circumference of the circle is . Since that is also equal to the circumference of the cone, the radius of the cone is . We also have that the slant height of the cone is . Therefore, we use the Pythagorean Theorem to calculate that the height of the coneis . The volume of the coneis -PCChessSolution 2 (Last Resort/Cheap)Using a ruler, measure a circle of radius 4 and cut out the circle and then the quarter missing. Then, fold it into a cone and measure the diameter to be 6cm . You can form a right triangle with sides 3, 4, and then through the Pythagorean theorem the height is found tobe . The volume of a cone is . Plugging in we findProblem11Ms. Carr asks her students to read any 5 of the 10 books on a reading list. Harold randomly selects 5 books from this list, and Betty does the same. What is the probability that there are exactly 2 books that they both select?SolutionWe don't care about which books Harold selects. We just care that Bettypicks books from Harold's list and that aren't on Harold's list.The total amount of combinations of books that Betty can selectis .There are ways for Betty to choose of the books that are on Harold's list.From the remaining books that aren't on Harold's list, thereare ways to choose of them.~quacker88Problem12The decimal representation of consists of a string of zeros after the decimal point, followed by a and then several more digits. How many zeros are in that initial string of zeros after the decimal point?Solution 1Now we do some estimation. Notice that , which meansthat is a little more than . Multiplying itwith , we get that the denominator is about . Notice that whenwe divide by an digit number, there are zeros before the first nonzero digit. This means that when we divide by the digitinteger , there are zeros in the initial string after the decimal point. -PCChessSolution 2First rewrite as . Then, we know that when we write this in decimal form, there will be 40 digits after the decimal point. Therefore, we just have to findthe number of digits in .and memming (alternatively use the factthat ),digits.Our answer is .Solution 3 (Brute Force)Just as in Solution we rewrite as We thencalculate entirely by hand, first doing then multiplying that product by itself, resulting in Because this is digits,after dividing this number by fourteen times, the decimal point is beforethe Dividing the number again by twenty-six more times allows a stringof zeroes to be formed. -OreoChocolateSolution 4 (Smarter Brute Force)Just as in Solutions and we rewrite as We can then look at the number of digits in powersof . , , , , ,, and so on. We notice after a few iterations that every power of five with an exponent of , the number of digits doesn't increase. This means should have digits since thereare numbers which are from to , or digits total. This means our expression can be written as , where is in therange . Canceling gives , or zeroes before the since the number should start on where the one would be in . ~aop2014 Solution 5 (Logarithms)Problem13Andy the Ant lives on a coordinate plane and is currently at facingeast (that is, in the positive -direction). Andy moves unit and thenturns degrees left. From there, Andy moves units (north) and thenturns degrees left. He then moves units (west) and againturns degrees left. Andy continues his progress, increasing his distance each time by unit and always turning left. What is the location of the point at which Andy makes the th leftturn?Solution 1You can find that every four moves both coordinates decrease by 2. Therefore, both coordinates need to decrease by two 505 times. You subtract, giving you theanswer of ~happykeeperProblem14As shown in the figure below, six semicircles lie in the interior of a regular hexagon with side length 2 so that the diameters of the semicircles coincide with the sides of the hexagon. What is the area of the shaded region — inside the hexagon but outside all of the semicircles?Solution 1Let point A be a vertex of the regular hexagon, let point B be the midpoint of the line connecting point A and a neighboring vertex, and let point C be the second intersection of the two semicircles that pass through point A. Then, , since B is the center of the semicircle with radius 1 that C lies on, , since B is the center of the semicircle with radius 1 that A lies on,and , as a regular hexagon has angles of 120,and is half of any angle in this hexagon. Now, using the sinelaw, , so . Since the angles in a triangle sum to 180, is also 60. Therefore, is an equilateral triangle with side lengths of 1.Since the area of a regular hexagon can be found with the formula , where is the side length of the hexagon, the area of this hexagonis . Since the area of an equilateral triangle can be foundwith the formula , where is the side length of the equilateral triangle,the area of an equilateral triangle with side lengths of 1 is . Since the area of a circle can be found with the formula , the area of a sixthof a circle with radius 1 is . In each sixth of the hexagon, thereare two equilateral triangles colored white, each with an area of , and onesixth of a circle with radius 1 colored white, with an area of . The rest of the sixth is colored gray. Therefore, the total area that is colored white in each sixthof the hexagon is , which equals , and the total areacolored white is , which equals . Since the area colored gray equals the total area of the hexagon minus the area colored white,the area colored gray is , whichequals .Solution 2First, subdivide the hexagon into 24 equilateral triangles with side length1:Now note that the entire shadedregion is just 6 times this part:The entire rhombus is just 2 equilatrial triangles with side lengths of 1, so it has an area of:The arc that is not included has an area of:Hence, the area ofthe shaded region in that section is For a final areaof:Problem15Steve wrote the digits , , , , and in order repeatedly from left to right, forming a list of digits, beginning He thenerased every third digit from his list (that is, the rd, th, th, digits from the left), then erased every fourth digit from the resulting list (that is, the th, th, th, digits from the left in what remained), and then erased every fifth digit from what remained at that point. What is the sum of the three digits that were then in the positions ?Solution 1After erasing every third digit, the list becomes repeated. After erasing every fourth digit from this list, the listbecomes repeated. Finally, after erasing every fifth digit from this list, the list becomes repeated. Since this list repeats every digits andsince are respectively in we have that the th, th, and st digits are the rd, th, and thdigits respectively. It follows that the answer is~dolphin7Problem16Bela and Jenn play the following game on the closed interval of the real number line, where is a fixed integer greater than . They take turns playing, with Bela going first. At his first turn, Bela chooses any real number in theinterval . Thereafter, the player whose turn it is chooses a real numberthat is more than one unit away from all numbers previously chosen by either player. A player unable to choose such a number loses. Using optimal strategy, which player will win the game?SolutionNotice that to use the optimal strategy to win the game, Bela must select themiddle number in the range and then mirror whatever number Jennselects. Therefore, if Jenn can select a number within the range, so can Bela. Jenn will always be the first person to run out of a number to choose, so theanswer is .Solution 2 (Guessing)First of all, realize that the value of should have no effect on the strategy at all. This is because they can choose real numbers, not integers, so even if is odd, for example, they can still go halfway. Similarly, there is no reason the strategy would change when .So we are left with (A) and (B). From here it is best to try out random numbers and try to find the strategy that will let Bela win, but if you can't find it, realize thatit is more likely the answer is since Bela has the first move and thus has more control.Problem17There are people standing equally spaced around a circle. Each person knows exactly of the other people: the people standing next to her or him, as well as the person directly across the circle. How many ways are there forthe people to split up into pairs so that the members of each pair know each other?SolutionLet us use casework on the number of diagonals.Case 1: diagonals There are ways: either pairs with , pairs with , and so on or pairs with , pairs with , etc.Case 2: diagonal There are possible diagonals to draw (everyone else pairs with the person next to them.Note that there cannot be 2 diagonals.Case 3: diagonalsNote that there cannot be a case with 4 diagonals because then there would have to be 5 diagonals for the two remaining people, thus a contradiction.Case 4: diagonals There is way to do this.Thus, in total there are possible ways. Problem18An urn contains one red ball and one blue ball. A box of extra red and blue balls lie nearby. George performs the following operation four times: he draws a ball from the urn at random and then takes a ball of the same color from the box and returns those two matching balls to the urn. After the four iterations the urn contains six balls. What is the probability that the urn contains three balls of each color?SolutionLet denote that George selects a red ball and that he selects a blue one. Now, in order to get balls of each color, he needs more of both and .There are 6cases:(wecan confirm that there are only since ). However we canclump , ,and together since they are equivalent by symmetry.andLet's find the probability that he picks the balls in the order of .The probability that the first ball he picks is red is .Now there are reds and blue in the urn. The probability that he picks red again is now .There are reds and blue now. The probability that he picks a blue is .Finally, there are reds and blues. The probability that he picks a blue is . So the probability that the case happensis . However, since the case is the exactsame by symmetry, case 1 has a probability of chance of happening.andLet's find the probability that he picks the balls in the order of .The probability that the first ball he picks is red is .Now there are reds and blue in the urn. The probability that he picks blue is .There are reds and blues now. The probability that he picks a red is . Finally, there are reds and blues. The probability that he picks a blue is .So the probability that the case happensis . However, since the case is the exactsame by symmetry, case 2 has a probability of chance of happening.andLet's find the probability that he picks the balls in the order of .The probability that the first ball he picks is red is .Now there are reds and blue in the urn. The probability that he picks blueis .There are reds and blues now. The probability that he picks a blue is .Finally, there are reds and blues. The probability that he picks a red is .So the probability that the case happensis . However, since the case is the exactsame by symmetry, case 3 has a probability of chance of happening.Adding up the cases, we have ~quacker88 Solution 2We know that we need to find the probability of adding 2 red and 2 blue balls insome order. There are 6 ways to do this, since there are ways to arrange in some order. We will show that the probability for each of these 6 ways is the same.We first note that the denominators should be counted by the same number. This number is . This is because 2, 3, 4, and 5 represent how many choices there are for the four steps. No matter what the stepinvolves numbers to choose from.The numerators are the number of successful operations. No matter the order, the first time a red is added will come from 1 choice and the second time will come from 2 choices, since that is how many reds are in the urn originally. Thesame goes for the blue ones. The numerator must equal . Therefore, the probability for each of the orderingsof is . There are 6 of these, so the total probabilityis .Solution 3First, notice that when George chooses a ball he just adds another ball of the same color. On George's first move, he either chooses the red or the blue witha chance each. We can assume he chooses Red(chance ), and then multiply the final answer by two for symmetry. Now, there are two red balls andone blue ball in the urn. Then, he can either choose another Red(chance ), in which case he must choose two blues to get three of each, withprobability or a blue for two blue and two red in the urn, withchance . If he chooses blue next, he can either choose a red then a blue, or ablue then a red. Each of these has a for total of . The total probability that he ends up with three red and three blueis . ~aop2014 Solution 4Let the probability that the urn ends up with more red balls be denoted . Since this is equal to the probability there are more blue balls, the probabilitythere are equal amounts is . the probability no more blues are chosen plus the probability only 1 more blue is chosen. The firstcase, .The second case, . Thus,the answer is .~JHawk0224Solution 5By conditional probability after 4 rounds we have 5 cases: RRRBBB, RRRRBB,RRBBBB, RRRRRB and RBBBBB. Thus the probability is . Put .~FANYUCHEN20020715Edited by KinglogicSolution 6Here X stands for R or B, and Y for the remaining color. After 3 rounds one can either have a 4+1 configuration (XXXXY), or 3+2 configuration (XXXYY). Theprobability of getting to XXXYYY from XXXYY is . Observe that the probability of arriving to 4+1 configuration is ( to get from XXY toXXXY, to get from XXXY to XXXXY). Thus the probability of arriving to 3+2configuration is also , and the answer isSolution 7We can try to use dynamic programming to solve this problem. (Informatics Olympiad hahaha)We let be the probability that we end up with red balls and blue balls. Notice that there are only two ways that we can end up with red balls and blue balls: one is by fetching a red ball from the urn when wehave red balls and blue balls and the other is by fetching a blue ball from the urn when we have red balls and blue balls.Then wehaveThen we start can with and try to compute .The answer is .Problem19In a certain card game, a player is dealt a hand of cards from a deckof distinct cards. The number of distinct (unordered) hands that can be dealt to the player can be written as . What is the digit ?Solution 1We're looking for the amount of ways we can get cards from a deck of ,which is represented by .We need to get rid of the multiples of , which will subsequently get rid of the multiples of (if we didn't, the zeroes would mess with the equation since you can't divide by 0), , leaves us with 17.Converting these into, we have~quacker88 Solution 2Since this number is divisible by but not , the last digits must be divisible by but the last digits cannot be divisible by . This narrows the options down to and .Also, the number cannot be divisible by . Adding up the digits, weget . If , then the expression equals , a multiple of . This would mean that the entire number would be divisible by , which is not what we want. Therefore, the only option is -PCChessSolution 3It is not hard to check that divides thenumber,As , using wehave .Thus , implying so the answer is .Solution 4As mentioned above,We can divide both sidesof by 10 to obtain which means is simply the units digit of the left-hand side. This valueisProblem20Let be a right rectangular prism (box) with edges lengths and ,together with its interior. For real , let be the set of points in -dimensional space that lie within a distance of some point . The volumeof can be expressed as ,where and are positive real numbers. What isSolutionSplit into 4 regions:1. The rectangular prism itself2. The extensions of the faces of3. The quarter cylinders at each edge of4. The one-eighth spheres at each corner ofRegion 1: The volume of is 12, soRegion 2: The volume is equal to the surface area of times . The surfacearea can be computed to be ,so .Region 3: The volume of each quarter cylinder is equal to . The sum of all such cylinders must equal times the sum of the edge lengths. This can be computed as , so the sum of the volumes of the quarter cylinders is , soRegion 4: There is an eighth of a sphere of radius at each corner. Since there are 8 corners, these add up to one full sphere of radius . The volume of thissphere is , so .Using these values,Problem21In square , points and lie on and , respectively, so that Points and lie on and , respectively, and points and lie on so that and . See the figure below. Triangle , quadrilateral ,quadrilateral , and pentagon each has area Whatis ?SolutionSince the total area is , the side length of square is . We see that since triangle is a right isosceles triangle with area 1, we can determinesides and both to be . Now, considerextending and until they intersect. Let the point of intersection be .We note that is also a right isosceles triangle with side and find it's area to be . Now, we notice that is also a rightisosceles triangle and find it's area to be . This is also equalto or . Since we are looking for , we want two times this. That gives .~TLiuSolution 2Since this is a geometry problem involving sides, and we know that is , we can use our ruler and find the ratio between and . Measuring(on the booklet), we get that is about inches and isabout inches. Thus, we can then multiply the length of by the ratioof of which we then get We take the square of that andget and the closest answer to that is . ~Celloboy (Note that this is just a strategy I happened to use that worked. Do not press your luck with this strategy, for it was a lucky guess)Solution 3Draw the auxiliary line . Denote by the point it intersects with , and by the point it intersects with . Last, denote by the segment , and by the segment . We will find two equations for and , and then solve for .Since the overall area of is ,and . In addition, the areaof .The two equations for and are then:Lengthof :Area of CMIF: .Substituting the first into the second,yieldsSolving for gives ~DrBSolution 4Plot a point such that and are collinear and extend line topoint such that forms a square. Extend line to meetline and point is the intersection of the two. The area of this square is equivalent to . We see that the area of square is , meaning each side is of length 2. The area of the pentagon is .Length , thus . Triangle is isosceles, and the area of this triangleis . Adding these two areas, we get . --OGBooger Solution 5 (HARD Calculation)We can easily observe that the area of square is 4 and its side length is 2 since all four regions that build up the square has area 1. Extend and let the intersection with be . Connect , and let the intersectionof and be . Notice that since the area of triangle is 1and , ,therefore . Let ,。
