数据结构实验报告图实验

合集下载

数据结构实验———图实验报告

数据结构实验报告目的要求１．掌握图的存储思想及其存储实现..２．掌握图的深度、广度优先遍历算法思想及其程序实现..３．掌握图的常见应用算法的思想及其程序实现..实验内容１．键盘输入数据;建立一个有向图的邻接表..２．输出该邻接表..3．在有向图的邻接表的基础上计算各顶点的度;并输出..4．以有向图的邻接表为基础实现输出它的拓扑排序序列..5．采用邻接表存储实现无向图的深度优先递归遍历..6．采用邻接表存储实现无向图的广度优先遍历..7．在主函数中设计一个简单的菜单;分别调试上述算法..源程序：主程序的头文件：队列#include <stdio.h>#include <stdlib.h>#define TRUE 1#define FALSE 0#define OK 1#define ERROR 0#define OVERFLOW -2typedef int QElemType;typedef struct QNode{ //队的操作QElemType data;struct QNode *next;}QNode;*QueuePtr;typedef struct {QueuePtr front;QueuePtr rear;}LinkQueue;void InitQueueLinkQueue &Q{ //初始化队列Q.front =Q.rear =QueuePtrmallocsizeofQNode;ifQ.front exitOVERFLOW; //存储分配失败Q.front ->next =NULL;}int EnQueueLinkQueue &Q;QElemType e //插入元素e为Q的新的队尾元素{QueuePtr p;p=QueuePtrmallocsizeofQNode;ifp exitOVERFLOW;p->data=e;p->next=NULL;Q.rear->next=p;Q.rear =p;return OK;}int DeQueueLinkQueue &Q;QElemType &e //删除Q的队头元素;用e返回其值{ ifQ.front ==Q.rear return ERROR;QueuePtr p;p=Q.front ->next;e=p->data;Q.front->next=p->next ;ifQ.rear==p Q.rear =Q.front ;freep;return OK;}主程序：#include <stdio.h>#include<stdlib.h>#include"duilie.h"#define TRUE 1#define FALSE 0#define Status int#define MAX_VERTEX_NUM 8 /*顶点最大个数*/#define VertexType char /*顶点元素类型*/enum BOOlean {False;True};BOOlean visitedMAX_VERTEX_NUM; //全局变量——访问标志数组typedef struct ArcNode{int adjvex;struct ArcNode *nextarc;int weight; /*边的权*/}ArcNode; /*表结点*/typedef struct VNode{ int degree;indegree;/*顶点的度;入度*/V ertexType data;ArcNode *firstarc;}VNode/*头结点*/;AdjListMAX_VERTEX_NUM;typedef struct{ AdjList vertices;int vexnum;arcnum;/*顶点的实际数;边的实际数*/}ALGraph;//建立图的邻接表void creat_linkALGraph *G{ int i;j;ArcNode *s;printf"请依次输入顶点数、边数：";scanf"%d%d";&G->vexnum;&G->arcnum;for i=0;i<G->vexnum;i++{ G->verticesi.data='A'+i;G->verticesi.firstarc=NULL;}for i=0;i<G->vexnum;{ printf"请输入顶点的数组坐标若退出;请输入-1：";scanf"%d";&i;ifi==-1 break;printf"请输入顶点所指向下一个顶点的数组坐标：";scanf"%d";&j;s=ArcNode *mallocsizeofArcNode;s->adjvex=j;s->nextarc=G->verticesi.firstarc;G->verticesi.firstarc=s;}}// 输出邻接表void visitALGraph G{ int i;ArcNode *p;printf"%4s%6s%18s\n";"NO";"data";"adjvexs of arcs";for i=0;i<G.vexnum;i++{printf"%4d%5c ";i;G.verticesi.data;forp=G.verticesi.firstarc;p;p=p->nextarcprintf"%3d";p->adjvex;printf"\n";}}// 计算各顶点的度及入度void cacuALGraph *G{ArcNode *p;int i;for i=0;i<G->vexnum;i++{G->verticesi.degree=0;G->verticesi.indegree=0;}//度与初度初始化为零for i=0;i<G->vexnum;i++forp=G->verticesi.firstarc;p;p=p->nextarc{G->verticesi.degree++;G->verticesp->adjvex.degree++;G->verticesp->adjvex.indegree++;}}void print_degreeALGraph G{int i;printf"\n Nom data degree indegree\n";for i=0;i<G.vexnum;i++printf"\n%4d%5c%7d%8d";i;G.verticesi.data;G.verticesi.degree;G.verticesi.indegree;printf"\n";}// 拓扑排序Status TopologiSortALGraph G{int i;count;top=0;stack50;ArcNode *p;cacu&G;print_degreeG;printf"\nTopologiSort is \n";fori=0;i<G.vexnum;i++ifG.verticesi.indegree stacktop++=i;count=0;whiletop=0{i=stack--top;if count==0 printf"%c";G.verticesi.data;else printf"-->%c";G.verticesi.data;count++;forp=G.verticesi.firstarc;p;p=p->nextarcif --G.verticesp->adjvex.indegreestacktop++=p->adjvex;}if count<G.vexnumreturnFALSE; else returnTRUE;}//在图G中寻找第v个顶点的第一个邻接顶点int FirstAdjVexALGraph G;int v{ifG.verticesv.firstarc return 0;else returnG.verticesv.firstarc->adjvex;}//在图G中寻找第v个顶点的相对于u的下一个邻接顶点int NextAdjVexALGraph G;int v;int u{ArcNode *p;p=G.verticesv.firstarc;whilep->adjvex=u p=p->nextarc; //在顶点v的弧链中找到顶点u ifp->nextarc==NULL return 0; //若已是最后一个顶点;返回0else returnp->nextarc->adjvex; //返回下一个邻接顶点的序号}//采用邻接表存储实现无向图的深度优先递归遍历void DFSALGraph G;int i{ int w;visitedi=True; //访问第i个顶点printf"%d->";i;forw=FirstAdjVexG;i;w;w=NextAdjVexG;i;wifvisitedw DFSG;w; //对尚未访问的邻接顶点w调用DFS}void DFSTraverseALGraph G{ int i;printf"DFSTraverse:";fori=0;i<G.vexnum;i++ visitedi=False; //访问标志数组初始化fori=0;i<G.vexnum;i++ifvisitedi DFSG;i; //对尚未访问的顶点调用DFS}//按广度优先非递归的遍历图G;使用辅助队列Q和访问标志数组visited void BFSTraverseALGraph G{int i;u;w;LinkQueue Q;printf"BFSTreverse:";fori=0;i<G.vexnum;i++ visitedi=False; //访问标志数组初始化InitQueueQ; //初始化队列fori=0;i<G.vexnum;i++ifvisitedi{visitedi=True; //访问顶点iprintf"%d->";i;EnQueueQ;i; //将序号i入队列whileQ.front ==Q.rear //若队列不空;继续{DeQueueQ;u; //将队头元素出队列并置为uforw=FirstAdjVexG;u;w;w=NextAdjV exG;u;wifvisitedw //对u的尚未访问的邻接顶点w进行访问并入队列{ visitedw=True;printf"%d->";w;EnQueueQ;w;}}}}void main{ALGraph G;int select;printf" 图的有关操作实验\n ";do{printf"\n1 创建一个有向图的邻接表 2 输出该邻接表\n";printf"3.输出该有向图的度和入度 4.输出该有向图拓扑排序序列\n";printf"5.创建一个无向图的邻接表 6.深度优先递归遍历该无向图\n";printf"7.广度优先遍历该无向图0.退出\n";printf"请输入选择：";scanf"%d";&select;switchselect{case 1:printf"\n创建一个有向图的邻接表：\n";creat_link&G;break;case 2:printf"\n输出该邻接表：\n";visitG;break;case 3:printf"\n输出该有向图的度和入度：\n";cacu&G;print_degreeG;break;case 4:printf"\n输出该有向图拓扑排序序列：\n";ifTopologiSortGprintf"Toposort is not success";break;case 5:printf"\n创建一个无向图的邻接表: \n";creat_link&G;break;case 6:printf"\n深度优先递归遍历该无向图: \n";DFSTraverseG;break;case 7:printf"\n广度优先遍历该无向图：\n";BFSTraverseG;break;case 0:break;default:printf"输入选项错误重新输入\n";}}whileselect;}运行结果截图：1.主菜单界面：2.创建一个有向图的领接表3.输出该邻接表4. 在有向图的邻接表的基础上计算各顶点的度;并输出..5. 输出它的拓扑排序序列6. 输出所建无向图的邻接表7. 深度优先递归遍历该无向图8. 广度优先遍历该无向图说明：本实验用的有向图是课本182页图7.28;无向图为课本168页图a实验总结这次的图的操作实验;与树的操作类似;但又比树复杂;包含更多的存储结构和遍历方法的操作;而且图的遍历需要沿着弧进行;以便输出弧上的信息..本实验中图的遍历采用邻接表的存储结构;在输入图的信息时;首先要画出图的邻接表信息..图有两种遍历的形式;一种为深度优先搜索;另一种为广度优先搜索..由于能力有限;没能实现图的深度非递归优先搜索;而是实现了图的深度递归优先搜索..本实验基本完成了图的操作;也学到了很多关于图的知识和算法..。

数据结构实验报告-答案.doc

数据结构实验报告-答案数据结构(C语言版)实验报告专业班级学号姓名实验1实验题目：单链表的插入和删除实验目的：了解和掌握线性表的逻辑结构和链式存储结构，掌握单链表的基本算法及相关的时间性能分析。

实验要求：建立一个数据域定义为字符串的单链表，在链表中不允许有重复的字符串；根据输入的字符串，先找到相应的结点，后删除之。

实验主要步骤：1、分析、理解给出的示例程序。

2、调试程序，并设计输入数据（如：bat，cat，eat，fat，hat，jat，lat，mat，#），测试程序的如下功能：不允许重复字符串的插入；根据输入的字符串，找到相应的结点并删除。

3、修改程序：（1）增加插入结点的功能。

（2）将建立链表的方法改为头插入法。

程序代码:#include“stdio.h“#include“string.h“#include“stdlib.h“#include“ctype. h“typedefstructnode//定义结点{chardata[10];//结点的数据域为字符串structnode*next;//结点的指针域}ListNode;typedefListNode*LinkList;//自定义LinkList单链表类型LinkListCreatListR1();//函数，用尾插入法建立带头结点的单链表LinkListCreatList(void);//函数，用头插入法建立带头结点的单链表ListNode*LocateNode();//函数，按值查找结点voidDeleteList();//函数，删除指定值的结点voidprintlist();//函数，打印链表中的所有值voidDeleteAll();//函数，删除所有结点，释放内存ListNode*AddNode();//修改程序：增加节点。

用头插法，返回头指针//==========主函数==============voidmain(){charch[10],num[5];LinkListhead;head=C reatList();//用头插入法建立单链表，返回头指针printlist(head);//遍历链表输出其值printf(“Deletenode(y/n):“);//输入“y“或“n“去选择是否删除结点scanf(“%s“,num);if(strcmp(num,“y“)==0||strcmp(num,“Y“)==0){printf(“PleaseinputDelete_data:“);scanf(“%s“,ch);//输入要删除的字符串DeleteList(head,ch);printlist(head);}printf(“Addnode?(y/n):“);//输入“y“或“n“去选择是否增加结点scanf(“%s“,num);if(strcmp(num,“y“)==0||strcmp(num,“Y“)==0){head=A ddNode(head);}printlist(head);DeleteAll(head);//删除所有结点，释放内存}//==========用尾插入法建立带头结点的单链表===========LinkListCreatListR1(void){charch[10];LinkListhead=(Li nkList)malloc(sizeof(ListNode));//生成头结点ListNode*s,*r,*pp;r=head;r->next=NULL;printf(“Input#toend“);//输入“#“代表输入结束printf(“\nPleaseinputN ode_data:“);scanf(“%s“,ch);//输入各结点的字符串while(strcmp(ch,“#“)!=0){pp=LocateNode(head,ch);//按值查找结点，返回结点指针if(pp==NULL){//没有重复的字符串，插入到链表中s=(ListNode*)malloc(sizeof(ListNode));strcpy(s->data,ch);r->next=s;r=s; r->next=NULL;}printf(“Input#toend“);printf(“PleaseinputNode_data:“);scanf(“%s“,ch);}returnhead;//返回头指针}//==========用头插入法建立带头结点的单链表===========LinkListCreatList(void){charch[100];LinkListhead,p;head =(LinkList)malloc(sizeof(ListNode));head->next=NULL;while(1){printf(“Input#toend“);printf(“PleaseinputNode_data:“);scanf(“%s“,ch);if(strcmp (ch,“#“)){if(LocateNode(head,ch)==NULL){strcpy(head->data,ch);p=(Li nkList)malloc(sizeof(ListNode));p->next=head;head=p;}}elsebreak;}retu rnhead;}//==========按值查找结点，找到则返回该结点的位置，否则返回NULL==========ListNode*LocateNode(LinkListhead,char*key){List Node*p=head->next;//从开始结点比较while(p!=NULL//扫描下一个结点returnp;//若p=NULL则查找失败，否则p指向找到的值为key的结点}//==========修改程序：增加节点=======ListNode*AddNode(LinkListhead){charch[10];ListNode*s,*pp ;printf(“\nPleaseinputaNewNode_data:“);scanf(“%s“,ch);//输入各结点的字符串pp=LocateNode(head,ch);//按值查找结点，返回结点指针printf(“ok2\n“);if(pp==NULL){//没有重复的字符串，插入到链表中s=(ListNode*)malloc(sizeof(ListNode));strcpy(s->data,ch);printf(“ok3\n“);s->next=head->next;head->next=s;}returnhead;}//==========删除带头结点的单链表中的指定结点=======voidDeleteList(LinkListhead,char*key){ListNode*p,*r,*q=hea d;p=LocateNode(head,key);//按key值查找结点的if(p==NULL){//若没有找到结点，退出printf(“positionerror”);exit(0);}while(q->next!=p)//p 为要删除的结点，q为p的前结点q=q->next;r=q->next;q->next=r->next;free(r);//释放结点}//===========打印链表=======voidprintlist(LinkListhead){ListNode*p=head->next;//从开始结点打印while(p){printf(“%s,“,p->data);p=p->next;}printf(“\n“);}//==========删除所有结点，释放空间===========voidDeleteAll(LinkListhead){ListNode*p=head,*r;while( p->next){r=p->next;free(p);p=r;}free(p);}实验结果：Input#toendPleaseinputNode_data:batInput#toendPleaseinputNode_data: catInput#toendPleaseinputNode_data:eatInput#toendPleaseinputNode_da ta:fatInput#toendPleaseinputNode_data:hatInput#toendPleaseinputNode_ data:jatInput#toendPleaseinputNode_data:latInput#toendPleaseinputNode _data:matInput#toendPleaseinputNode_data:#mat,lat,jat,hat,fat,eat,cat,bat ,Deletenode(y/n):yPleaseinputDelete_data:hatmat,lat,jat,fat,eat,cat,bat,Ins ertnode(y/n):yPleaseinputInsert_data:putposition:5mat,lat,jat,fat,eat,put,c at,bat,请按任意键继续...示意图：latjathatfateatcatbatmatNULLheadlatjathatfateatcatbatmatheadlatjatfateat putcatbatmatheadNULLNULL心得体会：本次实验使我们对链表的实质了解更加明确了，对链表的一些基本操作也更加熟练了。

数据结构实验报告-队列的操作

printf("元素入队列");
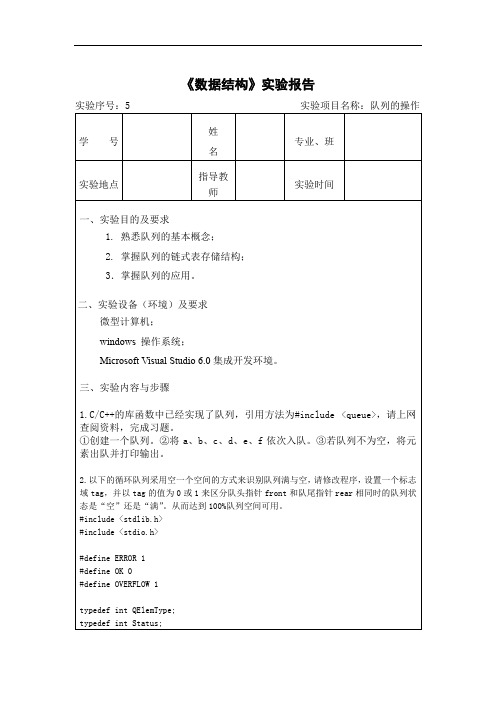
for(i=0 ; i<10; i++)
{
printf(" %d ",j);
EnQueue(S,j); //元素入队列
j++;
}
printf("\n元素出队列");
for(i=0 ; i<10; i++)
{
DeQueue(S,j); //元素出队列
printf(" %d ",j);
}
}
运行结果截图：
1.
四、分析与讨论
对上机实践结果进行分析，上机的心得体会。
五、教师评语
签名：
日期：
成绩
附源程序清单：
1.#include<iostream>
#include<queue>
using namespace std;
void main()
{
queue<char> cque;
char c;
typedef struct {
QElemType *base; // 动态分配存储空间
int front; // 头指针，若队列不空，指向队列头元素
int rear; // 尾指针，若队列不空， //指向队列尾元素的下一个位置
}SqQueue;
Status InitQueue (SqQueue &Q) { // 构造一个空队列Q
if ((Q.rear+1) % MAXQSIZE == Q.front)
return ERROR; //队列满
Q.base[Q.rear] = e;

数据结构试验报告-图的基本操作

中原工学院《数据结构》实验报告学院：计算机学院专业：计算机科学与技术班级：计科112姓名：康岩岩学号：201100814220 指导老师：高艳霞2012-11-22实验五图的基本操作一、实验目的1、使学生可以巩固所学的有关图的基本知识。

2、熟练掌握图的存储结构。

3、熟练掌握图的两种遍历算法。

二、实验内容[问题描述]对给定图，实现图的深度优先遍历和广度优先遍历。

[基本要求]以邻接表为存储结构，实现连通无向图的深度优先和广度优先遍历。

以用户指定的结点为起点，分别输出每种遍历下的结点访问序列。

【测试数据】由学生依据软件工程的测试技术自己确定。

三、实验前的准备工作1、掌握图的相关概念。

2、掌握图的逻辑结构和存储结构。

3、掌握图的两种遍历算法的实现。

四、实验报告要求1、实验报告要按照实验报告格式规范书写。

2、实验上要写出多批测试数据的运行结果。

3、结合运行结果，对程序进行分析。

【设计思路】【代码整理】#include "stdafx.h"#include <iostream>#include <malloc.h>using namespace std;typedef int Status;#define OK 1#define ERROR 0#define OVERFLOW -1#define MAX_SIZE 20typedef enum{DG,DN,UDG,UDN}Kind;typedef struct ArcNode{int adjvex; //顶点位置struct ArcNode *nextarc; //下一条弧int *info; //弧信息};typedef struct{char info[10]; //顶点信息ArcNode *fistarc; //指向第一条弧}VNode,AdjList[MAX_SIZE];typedef struct{AdjList vertices;int vexnum,arcnum; //顶点数，弧数int kind; //图的种类，此为无向图}ALGraph;//这是队列的节点，仅用于广度优先搜索typedef struct Node{int num;struct Node* next;};//队列的头和尾typedef struct{Node * front;Node *rear;}PreBit;int LocateV ex(ALGraph G,char info[]);//定位顶点的位置Status addArcNode(ALGraph &G,int adjvex); //图中加入弧Status CreatGraph(ALGraph&G);//创建图的邻接表Status DFSTraverse(ALGraph G);//深度优先搜索Status BFSTraverse(ALGraph G);//广度优先搜索Status DFS(ALGraph G,int v);//深度优先搜索中的数据读取函数，用于递归bool visited[MAX_SIZE]; // 访问标志数组//初始化队列Status init_q(PreBit&P_B){P_B.front=P_B.rear=(Node*)malloc(sizeof(Node));if(!P_B.front){exit(OVERFLOW);}P_B.front->next=NULL;}//将数据入队Status en_q(PreBit & P_B,int num){Node *p=(Node*)malloc(sizeof(Node));if(!p){exit(OVERFLOW);}p->num=num;p->next=NULL;P_B.rear->next=p;P_B.rear=p;return OK;}//出队Status de_q(PreBit & P_B){if(P_B.front==P_B.rear){return ERROR;}Node* p=P_B.front->next;P_B.front->next=p->next;if(P_B.rear==p){P_B.rear=P_B.front;}free(p);return OK;}Status CreatGraph(ALGraph&G){cout<<"请输入顶点数目和弧数目"<<endl;cin>>G.vexnum>>G.arcnum;//依次输入顶点信息for(int i=0;i<G.vexnum;i++){cout<<"请输入顶点名称"<<endl;cin>>G.vertices[i].info;G.vertices[i].fistarc=NULL;}//依次输入弧信息for(int k=1;k<=G.arcnum;k++){char v1[10],v2[10]; //用于表示顶点名称的字符数组int i,j; //表示两个顶点的位置BACK: //返回点cout<<"请输入第"<<k<<"条弧的两个顶点"<<endl;cin>>v1>>v2;i=LocateV ex(G,v1); //得到顶点v1的位置j=LocateV ex(G,v2); //得到顶点v2的位置if(i==-1||j==-1){ //头信息不存在则返回重输cout<<"不存在该节点!"<<endl;goto BACK; //跳到BACK 返回点}addArcNode(G,i); //将弧的顶点信息插入表中addArcNode(G,j);}return OK;}//倒序插入弧的顶点信息Status addArcNode(ALGraph &G,int adjvex){ArcNode *p; //弧节点指针p=(ArcNode*)malloc(sizeof(ArcNode));p->adjvex=adjvex;p->nextarc=G.vertices[adjvex].fistarc;//指向头结点的第一条弧G.vertices[adjvex].fistarc=p; //头结点的第一条弧指向p，即将p作为头结点的第一条弧return OK;}//定位顶点的位置int LocateV ex(ALGraph G,char info[]){for(int i=0;i<G.vexnum;i++){if(strcmp(G.vertices[i].info,info)==0){ //头结点名称与传入的信息相等，证明该头节点存在return i; //此时返回位置}}return -1;}//深度优先搜索Status DFSTraverse(ALGraph G){for(int v=0;v<G.vexnum;v++){visited[v]=false;}char v1[10];int i;BACK:cout<<"请输入首先访问的顶点"<<endl;cin>>v1;i=LocateV ex(G,v1);if(i==-1){cout<<"不存在该节点!"<<endl;goto BACK;}DFS(G,i);return OK;}//深度优先搜索递归访问图Status DFS(ALGraph G,int v){visited[v]=true;cout<<G.vertices[v].info<<" ";//输出信息ArcNode *p;p=G.vertices[v].fistarc; //向头节点第一条while(p) //当弧存在{if(!visited[p->adjvex]){DFS(G,p->adjvex); //递归读取}p=p->nextarc;}return OK;}//广度优先搜索Status BFSTraverse(ALGraph G){for(int v=0;v<G.vexnum;v++){visited[v]=false;}char v1[10];int v;BACK:cout<<"请输入首先访问的顶点"<<endl;cin>>v1;v=LocateV ex(G,v1);if(v==-1){cout<<"不存在该节点!"<<endl;goto BACK;}PreBit P_B;init_q(P_B);ArcNode *p;visited[v]=true;cout<<G.vertices[v].info<<" ";//输出信息en_q(P_B,v); //将头位置v入队while(P_B.front!=P_B.rear){//当队列不为空时，对其进行访问int w=P_B.front->next->num;//读出顶点位置de_q(P_B);//顶点已经访问过，将其出队列p=G.vertices[w].fistarc;//得到与顶点相关的第一条弧while(p){if(!visited[p->adjvex]){en_q(P_B,p->adjvex);//将弧入队，但不读取，只是将其放在队尾}p=p->nextarc;}}return OK;}int _tmain(int argc, _TCHAR* argv[]){ALGraph G;CreatGraph(G);cout<<"深度优先搜索图:"<<endl;DFSTraverse(G);cout<<endl;cout<<"广度优先搜索图:"<<endl;BFSTraverse(G);cout<<endl;system("pause");return 0;}。

数据结构实验报告--图

数据结构实验报告--图
数据结构实验报告--图
1、实验目的
本实验主要旨在通过实践操作，深入理解图这种数据结构的基本概念、性质和基本操作，掌握图的存储结构与常见算法。

2、实验环境
本次实验使用编程语言C++，在Windows平台下进行开发和运行。

3、实验内容
3.1 图的定义与基本概念
在本章中，我们将介绍图的基本概念，包括有向图与无向图、顶点与边、度与入度出度、连通性等。

3.2 图的存储结构
在本章中，我们将介绍图的几种存储结构，包括邻接矩阵、邻接表和十字链表，以及它们的优缺点和适用场景。

3.3 图的遍历
在本章中，我们将介绍图的两种常用的遍历算法，即深度优先搜索（DFS）和广度优先搜索（BFS），并分别给出它们的实现代码和应用场景。

3.4 最短路径
在本章中，我们将介绍图的最短路径问题，包括单源最短路径和全源最短路径。

我们将使用Dijkstra算法和Floyd-Warshall算法来解决这些问题，并给出它们的实现代码和应用场景。

3.5 最小树
在本章中，我们将介绍图的最小树问题，即找到一棵树使得树上的边的权值之和最小。

我们将使用Prim算法和Kruskal算法来解决这个问题，并给出它们的实现代码和应用场景。

4、实验步骤和结果
在本章中，我们将详细介绍实验的具体步骤，并给出实验结果的详细分析和说明。

5、实验总结
在本章中，我们将对整个实验进行总结，总结实验中遇到的问题、解决方案和经验教训。

6、附件
本实验报告所涉及的附件包括实验代码和运行结果的截图。

7、法律名词及注释
本文所涉及的法律名词和注释详见附件中的相关文件。

数据结构实验报告

A
B
C D
E F
G
主程序模块
结点单元模块构建先序二叉树模块
二叉树遍历模块
main
CreatBTree Preorder Inorder Postorde
程序的功能设计、数据结构设计及整体结构
设计合理；程序运行情况良好，算法说明清晰，理论分析与计算正确，实验数据无误熟练使用开辟工具，能够迅速准确的进行调
试、纠错和运行
良好的编程风格(缩进，注释，变量名、函
数名见名知意等，程序运行界面友好)
提交的电子文档及打印文档的书写、存放符
合规范化要求
能简明扼要地阐述设计的主要内容，能准确
流利地回答各种问题
端正的学习态度及认真刻苦程度等
30
20
10
10
20
10。

《数据结构》实验1实验报告

南京工程学院实验报告<班级>_<学号>_<实验X>.RAR文件形式交付指导老师。

一、实验目的1.熟悉上机环境，进一步掌握语言的结构特点。

2.掌握线性表的顺序存储结构的定义及实现。

3.掌握线性表的链式存储结构——单链表的定义及实现。

4.掌握线性表在顺序存储结构即顺序表中的各种基本操作。

5.掌握线性表在链式存储结构——单链表中的各种基本操作。

二、实验内容1.顺序线性表的建立、插入及删除。

2.链式线性表的建立、插入及删除。

三、实验步骤1.建立含n个数据元素的顺序表并输出该表中各元素的值及顺序表的长度。

2.利用前面的实验先建立一个顺序表L={21，23，14，5，56，17，31}，然后在第i个位置插入元素68。

3.建立一个带头结点的单链表，结点的值域为整型数据。

要求将用户输入的数据按尾插入法来建立相应单链表。

四、程序主要语句及作用程序1的主要代码(附简要注释)public struct sequenlist{public const int MAXSIZE=1024; /*最大值为1024*/public elemtype[] vec;public int len; /* 顺序表的长度 */public sequenlist( int n){vec=new elemtype[MAXSIZE ];len = n;}};class Program{static void Main(string[] args){sequenlist list1 = new sequenlist(5);for (int i = 0; i < 5; i++){list1.vec[i] = i;}for (int i = 0; i < 5; i++){Console.Write("{0}---", list1.vec[i]) ;}Console.WriteLine("\n");Console.WriteLine("表长：{0}\n",list1.len );Console.ReadKey();}}程序2的主要代码(附简要注释)public void insertlist(int i, int x){if (len >= MAXSIZE)throw new Exception("上溢"); /*长度大于最大值则抛出异常*/if (i < 1 || i > len + 1)throw new Exception("位置");/插入位置小于1或大于len+1则抛出插入位置错误的异常for (int j = len; j >= i; j--)vec[j] = vec[j - 1]; //注意第j个元素存在数组下标为j-1处vec[i - 1] = x;len++;}};class Program{static void Main(string[] args){sequenlist list2 = new sequenlist(7);list2.vec[0] = 21;list2.vec[1] = 23;list2.vec[2] = 14;list2.vec[3] = 5;list2.vec[4] = 56;list2.vec[5] = 17;list2.vec[6] = 31;Console.Write("请输入第i个位置插入元素：");int loc =Convert.ToInt32( Console.ReadLine());Console.Write("请输入第{0}个位置插入的元素：", loc);int ele = Convert.ToInt32(Console.ReadLine());Console.WriteLine("插入前的线性表：");for (int i = 0; i < list2.len ; i++){Console.Write("{0}---", list2.vec[i]);}Console.WriteLine("\n");list2.insertlist(loc, ele);Console.WriteLine("插入后的线性表：");for (int i = 0; i < list2.len ; i++){Console.Write("{0}---", list2.vec[i]);}Console.WriteLine("\n");Console.ReadKey();}}程序3的主要代码(附简要注释)class Node{private int num;public int Num{set { num = value; }/输入值get { return num; }/获得值}private Node next;public Node Next{set { next = value; }get { return next; }}}class Pp{static void Main(string[] args){Node head;Node tempNode, tempNode1;int i;head = new Node();Console.WriteLine("输入六项数据：\n");Console.Write("输入第1项数据:");head.Num = Convert.ToInt32(Console.ReadLine());head.Next = null;tempNode = head;for (i = 1; i < 6; i++){tempNode1 = new Node();Console.Write("输入第{0}项数据:",i+1);tempNode1.Num = Convert.ToInt32(Console.ReadLine());/插入项转换为整形数值 tempNode1.Next = null;tempNode.Next = tempNode1;tempNode = tempNode.Next;}Console.WriteLine("线性表:");tempNode = head;for (i = 0; i < 6; i++){Console.Write("{0}", tempNode.Num);if (i < 5){Console.Write("--");}tempNode = tempNode.Next;}Console.ReadKey();}}五、程序运行结果截图程序1程序2程序3六、收获，体会及问题(写得越详细、越个性化、越真实越好，否则我不知道你做这个实验的心路历程，也就无法充分地判断你是否是独立完成的这个实验、你是否在做这个实验时进行了认真仔细地思考、通过这个实验你是否在实践能力上得到了提高)这次试验刚开始做时完全不知道从哪下手，才刚上了几节课，对于线性表、链式表都不是理解的很透彻，不知道用哪个软件编写程序。

数据结构实验报告—图

《算法与数据结构》课程实验报告一、实验目的1.实现图的存储结构;2.通过图的相关算法实现，掌握其算法思想。

二、实验内容及要求1.无向带权图的存储结构(邻接矩阵、邻接表等自选)2.实现图的相关算法(1)计算指定顶点的度(2)图的深度优先遍历和广度优先遍历算法(3)分别使用Kruskal和Prim算法求解该图的最小生成树三、系统分析（1）数据方面：定义图的模板基类，在模板类定义中的数据类型参数表<class T,class E>中，T是定点数据的类型，E是边上所附数据的类型。

这个模板基类是按照带权无向图来定义的。

在该实验中定点的数据的类型为char型，边上所附数据的类型为int型。

且图的创建为无向图。

（2）功能方面：1.能够实现图的创建以及图的输出。

2.能够返回顶点在图中位置以及图中位置对应顶点的值。

3.返回当前图中的边数与顶点数。

4.返回输入边的权值。

5.能够插入一个顶点或插入顶点与之相关联的边。

6.删除边或删除顶点与之相关联的边。

7.计算顶点的度。

8.实现深度优先搜索、广度优先搜索遍历。

9.Kruskal算法、Prim算法生成最小生成树。

四、系统设计（1）设计的主要思路根据实验要求，首先确定图的存储结构，在根据存储结构编写模板类，并将需要实现的功能代码完善，再写出实现各个功能的菜单并进行调试。

由于在编写由图生成最小生成树中采用了最小堆以及并查集的算法，故需要将这两个个类的代码完成并进行调试。

最后将此次实验所涉及的类全部整理完全后，通过之前编写的菜单对功能进行依次调试，完成此次实验。

（2）数据结构的设计图是非线性结构，它的每一个顶点可以与多个其他顶点相关联，各顶点之间的关系是任意的。

可以用很多方法来存储图结构。

在此采用邻接矩阵来存储图结构。

首先将所有顶点的信息组织成一个顶点表，然后利用一个矩阵来表示各顶点之间的邻接关系，称为邻接矩阵。

下面针对带权无向图的邻接矩阵作出说明。

其中有一个类型为顺序表的顶点表向量VerticesList，用以存储顶点的信息，还有一个作为邻接矩阵使用的二维数组Edge，用以存储图中的边，其矩阵元素个数取决于顶点个数，与边数无关。

数据结构--图的实验报告

图的实验报告班级：电子091 学号：0908140620 姓名：何洁编号：19（一）实验要求创建一个图。

能够实现图的输入，插入顶点和边，利用队列进行深度和广度遍历。

（二）需求分析功能：1，输入图的信息；2，插入一个顶点；3插入一个边；4，删除一个顶点；5，删除一个边；6，深度优先遍历；7，广度优先遍历；8退出。

（三）概要设计本程序采用的是模板类，抽象数据类型有：T,E。

类：template <class T,class E>class Graphmtx {friend istream & operator>>(istream& in,Graphmtx<T, E>& G);friend ostream & operator<<(ostream& out, Graphmtx<T, E>& G);//输出public:Graphmtx(int sz=30, E max=0); //构造函数~Graphmtx () //析构函数{ delete []VerticesList; delete []Edge; }T getValue (int i) {//取顶点i 的值, i 不合理返回0return i >= 0 && i <= numVertices ?V erticesList[i] : NULL;}E getWeight (int v1, int v2) { //取边(v1,v2)上权值return v1 != -1 && v2 != -1 ? Edge[v1][v2] : 0;}int NumberOfEdges(){return numEdges;} //返回当前边数int NumberOfVertices(){return numVertices;} //返回当前顶点int getFirstNeighbor (int v);//取顶点v 的第一个邻接顶点int getNextNeighbor (int v, int w);//取v 的邻接顶点w 的下一邻接顶点bool insertVertex (const T& vertex);//插入顶点vertexbool insertEdge (int v1, int v2, E cost);//插入边(v1, v2),权值为costbool removeVertex (int v);//删去顶点v 和所有与它相关联的边bool removeEdge (int v1, int v2);//在图中删去边(v1,v2)int getVertexPos (T vertex) {//给出顶点vertex在图中的位置for (int i = 0; i < numVertices; i++)if (VerticesList[i] == vertex) return i;return -1;}//int numVertexPos(T vertex);private:int maxVertices;int numEdges;int numVertices;T *VerticesList; //顶点表E **Edge; //邻接矩阵const E maxWeight;};（四）详细设计函数通过调用图类中的函数实现一些功能。

数据结构实验报告及心得体会

数据结构实验报告及心得体会一、概述：介绍本次实验的目的、背景以及所使用的实验环境和工具。

本次实验旨在通过实际操作，深入理解和掌握数据结构的原理及应用。

实验背景源于课程学习的理论知识与实际应用相结合的需求，通过实验操作，期望能够将课堂所学的数据结构知识更好地运用到实际编程和解决现实问题中。

本次实验所使用的实验环境为先进的计算机实验室，配备了高性能的计算机硬件和丰富的软件开发工具。

为了完成实验，我使用了Java编程语言，并结合Eclipse开发环境进行编程和调试。

我还参考了相关的数据结构专业书籍和在线资源，以便更好地理解和应用数据结构知识。

在实验过程中，我严格按照实验指导书的步骤进行操作，并认真记录了实验数据和结果。

通过本次实验，我深刻体会到了数据结构的重要性，也对数据结构的实现和应用有了更深入的了解。

二、实验内容：分别介绍线性数据结构（线性表）、非线性数据结构（二叉树、图）的实验内容，包括其实现方法、操作过程等。

每个实验都包含具体的实验目的和预期结果。

三、实验过程及结果分析：详细描述实验过程，包括实验步骤的执行情况，遇到的问题及解决方法。

对实验结果进行展示，并进行数据分析和结论。

这部分是实验报告的核心部分，体现了学生的实践能力和问题解决能力。

四、心得体会：分享在实验过程中的心得体会，包括遇到的困难、收获，对数据结构的理解与认识提升，以及实验过程中的团队协作和学习体验等。

这部分内容可以体现出学生的思考深度和学习的主观感受。

五、总结与展望：对本次实验报告进行总结，并对未来数据结构与算法的学习提出展望和建议。

这部分内容可以帮助学生梳理所学知识，明确未来的学习方向。

数据结构试验报告一海龟作图（样例5）

数据结构试验报告一海龟作图（样例5）第一篇：数据结构试验报告一海龟作图实验报告:海龟作图题目：设计一个能够实现海龟抽象数据类型Turtle。

海龟作图的抽象数据类型的定义为：ADT Turtle{ 数据对象：D={ai |ai∈CharSet，i=1,2,3，…，n n>=0} 数据关系：R1={|ai-1,ai∈D,ai-1void StartTurtleGraphics(char name ,int num1,int num2)操作结果：显示作图窗口并在窗口内写出本人的姓名name、上机号num1，实习题号num2 void StartTurtle(new Turtle &raphael,aPoint startPos)操作结果：初始化了一个新海龟，定位在startPos，并置画笔状态为落笔、龟头朝向为0，步进的尺寸因子为1。

void PenUp（newturtle &raphael）初始条件：海龟已存在。

操作结果：设置画笔状态为抬笔。

从此时起，海龟在屏幕上移动时将不在屏幕上作图。

void PenDown（newturtle &raphael）初始条件：海龟已存在。

操作结果：设置画笔状态为落笔。

从此时起，海龟在屏幕上移动时将在屏幕上作图。

int TurtleHeading（newturtle &raphael，int single）初始条件：海龟已存在。

操作结果：返回海龟头当前朝向放角度single。

aPoint * TurtlePos（newturtle &raphael）初始条件：海龟已存在。

操作结果：返回海龟头当前位置。

void Move（newturtle &raphael，float steps）初始条件：海龟已存在。

操作结果：依照海龟头的当前朝向，向前移动steps步。

void Turn（newturtle &raphael，float size）初始条件：海龟已存在。

数据结构图实验报告

1. 了解熟知图的定义和图的基本术语，掌握图的几种存储结构。

2. 掌握邻接矩阵和邻接表定义及特点，并通过实例解析掌握邻接矩阵和邻接表的类型定义。

3. 掌握图的遍历的定义、复杂性分析及应用，并掌握图的遍历方法及其基本思想。

1. 建立无向图的邻接矩阵2. 图的深度优先搜索3. 图的广度优先搜索建立无向图的邻接矩阵：源代码：#include "stdio.h"#include "stdlib.h"#define MAXSIZE 30typedef structchar vertex[MAXSIZE];//顶点为字符型且顶点表的长度小于MAXSIZEint edges[MAXSIZE][MAXSIZE];//边为整形且 edges 为邻近矩阵}MGraph;//MGraph 为采用邻近矩阵存储的图类型void CreatMGraph(MGraph *g,int e,int n){//建立无向图的邻近矩阵 g- >egdes，n 为顶点个数， e 为边数int i,j,k;printf("Input data of vertexs(0~n-1):\n");for(i=0;i<n;i++)g- >vertex[i]=i; //读入顶点信息for(i=0;i<n;i++)for(j=0;j<n;j++)g- >edges[i][j]=0; //初始化邻接矩阵for(k=1;k<=e;k++)//输入 e 条边{printf("Input edges of(i,j):");scanf("%d,%d",&i,&j);g- >edges[i][j]=1;g- >edges[j][i]=1;}void main(){int i,j,n,e;MGraph *g; //建立指向采用邻接矩阵存储图类型指针g=(MGraph*)malloc(sizeof(MGraph));//生成采用邻接举证存储图类型的存储空间printf("Input size of MGraph:"); //输入邻接矩阵的大小scanf("%d",&n);printf("Input number of edge:"); //输入邻接矩阵的边数scanf("%d",&e);CreatMGraph(g,e,n); //生成存储图的邻接矩阵printf("Output MGraph:\n");//输出存储图的邻接矩阵for(i=0;i<n;i++){for(j=0;j<n;j++)printf("%4d",g- >edges[i][j]);printf("\n");}}1) 源代码：#include "stdio.h"#include "stdlib.h"#define MAXSIZE 30typedef struct node//邻接表结点{int adjvex;//邻接点域struct node *next;//指向下一个邻接边结点的指针域}EdgeNode; //邻接表结点类型typedef struct vnode//顶点表结点{int vertex;//顶点域EdgeNode *firstedge; // 指向邻接表第一个邻接边节点的指针域}VertexNode;//顶点表结点类型void CreatAdjlist(VertexNode g[],int e,int n){//建立无向图的邻接表， n 为顶点数， e 为边数， g[]存储 n 个顶点表结点EdgeNode *p;int i,j,k;printf("Input data of vetex(0~n-1);\n");for(i=0;i<n;i++)//建立有 n 个顶点的顶点表{g[i].vertex=i; //读入顶点 i 信息g[i].firstedge=NULL; //初始化指向顶点 i 的邻接表表头指针}for (k=1;k<=e;k++)//输入 e 条边{printf("Input edge of(i,j):");scanf("%d,%d",&i,&j);p=(EdgeNode*)malloc(sizeof(EdgeNode));p- >adjvex=j; //在顶点 vi 的邻接表中添加邻接点为j 的结点p- >next=g[i].firstedge; //插入是在邻接表表头进行的g[i].firstedge=p;p=(EdgeNode*)malloc(sizeof(EdgeNode));p- >adjvex=i; //在顶点 vj 的邻接表中添加邻接点为 i 的结点p- >next=g[j].firstedge; //插入是在邻接表表头进行的g[j].firstedge=p;}}int visited[MAXSIZE]; //MAXSIZE 为大于或者等于无向图顶点个数的常量void DFS(VertexNode g[],int i){EdgeNode *p;printf("%4d",g[i].vertex); //输出顶点 i 信息，即访问顶点 i visited[i]=1;p=g[i].firstedge; //根据顶点 i 的指针 firstedge 查找其邻接表的第一个邻接边结点while(p!=NULL){if(!visited[p- >adjvex]) //如果邻接的这个边结点未被访问过DFS(g,p- >adjvex); //对这个边结点进行深度优先搜索p=p- >next; //查找顶点 i 的下一个邻接边结点}}void DFSTraverse(VertexNode g[],int n){//深度优先搜索遍历以邻接表存储的图，其中 g 为顶点数， n 为顶点个数int i;for(i=0;i<n;i++)visited[i]=0; //访问标志置 0for(i=0;i<n;i++)//对 n 个顶点的图查找未访问过的顶点并由该顶点开始遍历if(!visited[i]) //当 visited[i]等于 0 时即顶点 i 未访问过DFS(g,i); //从未访问过的顶点 i 开始遍历}void main(){int e,n;VertexNode g[MAXSIZE]; //定义顶点表结点类型数组 gprintf("Input number of node:\n");//输入图中节点个数边的个数scanf("%d",&n);printf("Input number of edge:\n");//输入图中边的个数scanf("%d",&e);printf("Make adjlist:\n");CreatAdjlist(g,e,n); //建立无向图的邻接表printf("DFSTraverse:\n");DFSTraverse(g,n); //深度优先遍历以邻接表存储的无向图printf("\n");}1) 源代码：#include "stdio.h"#include "stdlib.h"#define MAXSIZE 30typedef struct node1//邻接表结点{int adjvex; //邻接点域struct node1 *next;//指向下一个邻接边结点的指针域}EdgeNode; //邻接表结点类型typedef struct vnode//顶点表结点{int vertex;//顶点域EdgeNode *firstedge; // 指向邻接表第一个邻接边结点的指针域}VertexNode; //顶点表结点类型void CreatAdjlist(VertexNode g[],int e,int n){ //建立无向图的邻接表，n 为顶点数，e 为边数，g[]存储 n 个顶点表结点EdgeNode *p;int i,j,k;printf("Input data of vetex(0~n-1):\n");for(i=0;i<n;i++) //建立有 n 个顶点的顶点表{g[i].vertex=i; //读入顶点 i 信息g[i].firstedge=NULL; //初始化指向顶点 i 的邻接表表头指针}for(k=1;k<=e;k++) //输入 e 条边{printf("Input edge of(i,j):");scanf("%d,%d",&i,&j);p=(EdgeNode *)malloc(sizeof(EdgeNode));p- >adjvex=j;//在定点 vi 的邻接表中添加邻接点为 j 的结点p- >next=g[i].firstedge;//插入是在邻接表表头进行的g[i].firstedge=p;p=(EdgeNode *)malloc(sizeof(EdgeNode));p- >adjvex=i; //在顶点 vj 的邻接表中添加邻接点为 i 的结点p- >next=g[j].firstedge; //插入是在邻接表表头进行的g[j].firstedge=p;}}typedef struct node{int data;struct node *next;}QNode; //链队列结点的类型typedef struct{QNode *front,*rear; //将头、尾指针纳入到一个结构体的链队列}LQueue; //链队列类型void Init_LQueue(LQueue **q) //创建一个带头结点的空队列{QNode *p;*q=(LQueue *)malloc(sizeof(LQueue)); //申请带头、尾指针的链队列p=(QNode *)malloc(sizeof(QNode)); //申请链队列的头结点p- >next=NULL;//头结点的 next 指针置为空(*q)- >front=p; //队头指针指向头结点(*q)- >rear=p; //队尾指针指向头结点}int Empty_LQueue(LQueue *q) //判队空{if(q- >front==q- >rear) //队为空return 1;elsereturn 0;}void In_LQueue(LQueue *q,int x) //入队{QNode *p;p=(QNode *)malloc(sizeof(QNode)); //申请新链队列结点p- >data=x;p- >next=NULL; //新结点作为队尾结点时其 next 域为空q- >rear- >next=p; //将新结点*p 链到原队尾结点之后q- >rear=p; //使队尾指针指向新的队尾结点*p}void Out_LQueue(LQueue *q,int *x) //出队{QNode *p;if(Empty_LQueue(q))printf("Queue is empty!\n");//对空，出队失败else{p=q- >front- >next; //指针 p 指向链队列第一个数据结点(即对头结点)q- >front- >next=p- >next;//头结点的 next 指针指向链队列第二个数据结点(即删除第一个数据结点)*x=p- >data; //将删除的对头结点数据经由 x 返回free(p);if(q- >front- >next==NULL) //出队后队为空，则置为空q- >rear=q- >front;}}int visited[MAXSIZE]; //MAXSIZE 为大于或者等于无向图顶点个数的常量void BFS(VertexNode g[],LQueue *Q,int i){//广度优先搜索遍历邻接表存储的图，g 为顶点表，Q 为队指针，i 为第 i 个顶点int j,*x=&j;EdgeNode *p;printf("%4d",g[i].vertex); //输出顶点 i 信息，即访问顶点 i visited[i]=1; //置顶点 i 为访问过标志In_LQueue(Q,i); //顶点 i 入队 Qwhile(!Empty_LQueue(Q)) //当队 Q 非空时{Out_LQueue(Q,x); //对头顶点出队并送 j (暂记为顶点 j )p=g[j].firstedge;// 根据顶点 j 的表头指针查找其邻接表的第一个邻接边结点while(p!=NULL){if(!visited[p- >adjvex])//如果邻接的这个边结点未被{printf("%4d",g[p- >adjvex].vertex); // 输出这个邻接边结点的顶点信息visited[p- >adjvex]=1; //置该邻接边结点为访问过标志In_LQueue(Q,p- >adjvex); //将该邻接边结点送人队 Q}p=p- >next;//在顶点j 的邻接表中查找j 的下一个邻接边结点}}}void main(){int e,n;VertexNode g[MAXSIZE];//定义顶点表结点类型数组 gLQueue *q;printf("Input number of node:\n"); //输入图中结点个数scanf("%d",&n);printf("Input number of edge:\n");//输入图中边的个数scanf("%d",&e);printf("Make adjlist:\n ");CreatAdjlist(g,e,n);//建立无向图的邻接表Init_LQueue(&q);//队列 q 初始化printf("BFSTraverse:\n");BFS(g,q,0); //广度优先遍历以邻接表存储的无向图printf("\n");}1．通过本次试验让我对图的遍历以及图的深度和广度优先搜索有了更深刻的记忆和理解，将课本理论的知识得以实践。

数据结构实验报告

数据结构实验报告本次数据结构实验的主要内容是关于树的相关操作，包括树的创建、遍历、查找等。

通过实验，我们将对树的基本概念和操作进行深入了解，并掌握相关算法的实现和应用。

首先，我们将介绍树的创建和基本操作。

树是一种非线性数据结构，由节点和边组成，具有层次关系。

在实验中，我们通过数组和链表两种方式来创建树。

数组表示法是将树的节点按照从上到下、从左到右的顺序存储在一维数组中，通过计算节点的下标来实现对树的操作。

链表表示法则是利用指针来表示节点之间的关系，实现树的各种操作。

在创建树的过程中，我们需要考虑节点的插入、删除和修改等操作，以及树的遍历方式，包括前序遍历、中序遍历和后序遍历。

其次，我们将介绍树的查找操作。

在实际应用中，我们经常需要对树进行查找操作，以找到特定的节点或者进行相关的数据处理。

在本次实验中，我们将学习如何实现对树的查找操作，包括深度优先搜索（DFS）和广度优先搜索（BFS）两种方式。

通过这些查找算法，我们可以高效地找到树中的特定节点，并进行相应的处理。

最后，我们将进行树的应用实例分析。

树作为一种重要的数据结构，在实际应用中有着广泛的应用。

我们将通过实例分析，介绍树在各种领域的应用，包括文件系统、数据库索引、网络路由等方面。

通过这些实例，我们可以更好地理解树的重要性和实际应用。

总之，本次数据结构实验涉及了树的创建、遍历、查找和应用等方面，通过实验，我们将对树的相关概念和操作有更深入的理解，并掌握相关算法的实现和应用。

希望通过本次实验，能够对数据结构有更深入的认识，为今后的学习和应用打下良好的基础。

数据结构实验报告图的存储

数据结构实验报告图的存储数据结构图实验报告一、实验目的和要求（1）掌握图的相关概念，包括图，有向图，无向图，完全图，子图，连通图，度，入度，出度，简单回路和环等定义。

（2）重点掌握图的各种存储结构，包括邻接矩阵和邻接表等。

（3）重点掌握图的基本运算，包括创建图，输出图，深度优先遍历，广度优先遍历等。

（4）掌握图的其他运算，包括最小生成树，最短路径，拓扑排序和关键路径等算法。

（5）灵活运用图这种数据结构解决一些综合应用问题。

二、实验内容和方法(1)实验内容：1、编写一个程序algo8-1.cpp，实现不带权图和带权图的邻接矩阵与邻接表的相互转换算法、输出邻接矩阵与邻接表的算法，并在此基础上设计一个程序exp8-1.cpp实现如下功能：①建立如图1所示的有向图G的邻接矩阵，并输出；②由有向图G的邻接矩阵产生邻接表，并输出；③再由②的邻接表产生对应的邻接矩阵，并输出。

图12、编写一个程序algo8-2.cpp，实现图的遍历运算，并在此基础上设计一个程序exp8-2.cpp完成如下功能：①输出图1所示的有向图G从顶点0开始的深度优先遍历序列（递归算法）；②输出图1所示的有向图G从顶点0开始的深度优先遍历序列（非递归算法）；③输出图1所示的有向图G从顶点0开始的广度优先遍历序列。

3、设计一个程序exp8-3.cpp,采用邻接表存储图，并输出图8.1（a）中从指定顶点1出发的所有深度优先遍历序列。

(2)实验方法：1、综合运用课本所学的知识，用不同的算法实现在不同的程序功能。

2、结合指导老师的指导，解决程序中的问题，正确解决实际中存在的异常情况，逐步改善功能。

3、根据实验内容，编译程序。

三、实验环境：Windows 7，Visual C++6.0三、实验过程描述文件graph.h中定义了图的邻接矩阵表示类型和邻接表表示类型，该头文件在以下三个实验中都会使用到。

其代码如下：#ifndef GRAPH_H_INCLUDED#define GRAPH_H_INCLUDEDtypedef int InfoType;#define MAXV 100 //最大顶点个数#define INF 32767 //INF表示无限大//以下定义邻接矩阵类型typedef struct{int no;InfoType info;}VertexType;typedef struct{int edges[MAXV][MAXV];int n,e;VertexType vexs[MAXV];}MGraph;//以下定义邻接表类型typedef struct ANode{int adjvex;struct ANode* nextarc;InfoType info;}ArcNode;typedef int Vertex;typedef struct VNode{Vertex data;实验①源程序。

数据结构实验报告(实验)

深圳大学实验报告课程名称：数据结构实验与课程设计实验项目名称：实验一：顺序表的应用学院：计算机与软件学院专业：指导教师： **报告人：文成学号： ********** 班级： 5 实验时间： 2012-9-17实验报告提交时间： 2012-9-24教务部制一、实验目的与要求：目的：1.掌握线性表的基本原理2.掌握线性表地基本结构3.掌握线性表地创建、插入、删除、查找的实现方法要求：1.熟悉C++语言编程2.熟练使用C++语言实现线性表地创建、插入、删除、查找的实现方法二、实验内容：Problem A: 数据结构——实验1——顺序表例程Description实现顺序表的创建、插入、删除、查找Input第一行输入顺序表的实际长度n第二行输入n个数据第三行输入要插入的新数据和插入位置第四行输入要删除的位置第五行输入要查找的位置Output第一行输出创建后，顺序表内的所有数据，数据之间用空格隔开第二行输出执行插入操作后，顺序表内的所有数据，数据之间用空格隔开第三行输出执行删除操作后，顺序表内的所有数据，数据之间用空格隔开第四行输出指定位置的数据Sample Input611 22 33 44 55 66888 352Sample Output11 22 33 44 55 6611 22 888 33 44 55 6611 22 888 33 55 6622HINT第i个位置是指从首个元素开始数起的第i个位置，对应数组内下标为i-1的位置Problem B: 数据结构——实验1——顺序表的数据交换Description实现顺序表内的元素交换操作Input第一行输入n表示顺序表包含的·n个数据第二行输入n个数据，数据是小于100的正整数第三行输入两个参数，表示要交换的两个位置第四行输入两个参数，表示要交换的两个位置Output第一行输出创建后，顺序表内的所有数据，数据之间用空格隔开第二行输出执行第一次交换操作后，顺序表内的所有数据，数据之间用空格隔开第三行输出执行第二次交换操作后，顺序表内的所有数据，数据之间用空格隔开注意加入交换位置的合法性检查，如果发现位置不合法，输出error。

数据结构实验报告--图

.数据结构实验报告图一、实验目的1、熟悉图的结构和相关算法。

二、实验内容及要求1、编写创建图的算法。

2、编写图的广度优先遍历、深度优先遍历、及求两点的简单路径和最短路径的算法。

三、算法描述1、图的邻接表存储表示：对图的每个顶点建立一个单链表，第i个单链表表示所有依附于第i个点的边（对于有向图表示以该顶点为尾的弧）；链表的每个节点存储两个信息，该弧指向的顶点在图中的位置（adjvex）和指向下一条弧的指针（nextarc）。

每个连表的头结点存储顶点的数据：顶点信息（data）和指向依附于它的弧的链表域。

存储表示如下：typedef struct ArcNode {int adjvex; // 该弧所指向的顶点的位置struct ArcNode *nextarc;// 指向下一条弧的指针// InfoType *info; // 该弧相关信息的指针} ArcNode;typedef struct VNode {char data; // 顶点信息int data2;int sngle;ArcNode *firstarc;// 指向第一条依附该顶点的弧} VNode, AdjList[MAX_NUM];typedef struct {AdjList vertices;int vexnum, arcnum;int kind; // 图的种类标志} ALGraph;2、深度优先搜索：假设初始态是图中所有定点未被访问，从图中的某个顶点v开始，访问此顶点，然后依次从v的未访问的邻接点出发深度优先遍历，直至途中所有和v有相同路径的点都被访问到；若图中仍有点未被访问，则从图中另选一个未被访问的点作为起点重复上述过程，直到图中所有点都被访问到。

为了便于区分途中定点是否被访问过，需要附设一个访问标致数组visited [0..n-1]，将其初值均设为false，一旦某个顶点被访问，将对应的访问标志赋值为true。

2、广度优先搜索：假设初始态是图中所有顶点未被访问，从图中的某个顶点v开始依次访问v的各个未被访问的邻接点，然后分别从这些邻接点出发以此访问他们的邻接点，并使“先被访问的邻接顶点”先于“后被访问的邻接顶点”被访问，直至图中所有已被访问过的顶点的邻接顶点都被访问。

数据结构实验报告图

数据结构实验报告图一、实验目的本次实验的主要目的是深入理解和掌握图这种数据结构的基本概念、存储结构和相关算法，并通过实际编程实现来提高对图的操作和应用能力。

二、实验环境本次实验使用的编程语言为C+＋，开发工具为Visual Studio 2019。

三、实验内容（一）图的存储结构1、邻接矩阵邻接矩阵是用一个二维数组来表示图中顶点之间的关系。

如果顶点i 和顶点 j 之间有边相连，则数组中对应的元素值为 1；否则为 0。

这种存储结构简单直观，适用于顶点数较少且边数较多的稠密图。

2、邻接表邻接表是为图的每个顶点建立一个单链表，链表中存储的是与该顶点相邻的顶点信息。

这种存储结构在存储空间上比较节省，适用于顶点数较多且边数较少的稀疏图。

（二）图的遍历算法1、深度优先遍历（DepthFirst Search，简称 DFS）从图中的某个顶点出发，沿着一条路径尽可能深地访问顶点，直到无法继续前进，然后回溯到上一个未完全访问的顶点，继续进行深度优先搜索。

2、广度优先遍历（BreadthFirst Search，简称 BFS）从图中的某个顶点出发，先访问其所有相邻的顶点，然后再依次访问这些相邻顶点的相邻顶点，以此类推，逐层向外扩展。

（三）图的最短路径算法1、迪杰斯特拉（Dijkstra）算法用于求解单源最短路径问题，即从一个给定的源顶点到图中其他所有顶点的最短路径。

2、弗洛伊德（Floyd）算法用于求解任意两个顶点之间的最短路径。

四、实验步骤（一）邻接矩阵的实现｀｀｀cppinclude ＜iostream>using namespace std;const int MAX_VERTEX_NUM ＝ 100;class Graph ｛private:int vertexNum;int edgeNum;int adjMatrixMAX_VERTEX_NUMMAX_VERTEX_NUM;public:Graph(int vNum) ｛vertexNum ＝ vNum;edgeNum ＝ 0;for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛adjMatrixij ＝ 0;｝｝｝void addEdge(int i, int j) ｛if （i ＞＝ 0 ＆＆ i ＜ vertexNum ＆＆ j ＞＝ 0 ＆＆ j ＜ vertexNum) ｛adjMatrixij ＝ 1;adjMatrixji ＝ 1;edgeNum+＋；｝｝void printGraph(）｛for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛cout ＜＜ adjMatrixij ＜＜＂＂；｝cout ＜＜ endl;｝｝｝；int main(）｛Graph g(5)；gaddEdge(0, 1)；gaddEdge(0, 2)；gaddEdge(1, 2)；gaddEdge(2, 3)；gaddEdge(3, 4)；gprintGraph(）；return 0;｝｀｀｀（二）邻接表的实现｀｀｀cppinclude ＜iostream>include ＜vector>using namespace std;const int MAX_VERTEX_NUM ＝ 100; class Graph ｛private:int vertexNum;vector<int> adjListMAX_VERTEX_NUM;public:Graph(int vNum) ｛vertexNum ＝ vNum;｝void addEdge(int i, int j) ｛if （i ＞＝ 0 ＆＆ i ＜ vertexNum ＆＆ j ＞＝ 0 ＆＆ j ＜ vertexNum) ｛adjListipush_back(j)；adjListjpush_back(i)；｝｝void printGraph(）｛for （int i ＝ 0; i ＜ vertexNum; i+＋）｛cout ＜＜ i ＜＜＂：＂；for （int j ＝ 0; j ＜ adjListisize(）； j+＋）｛cout ＜＜ adjListij ＜＜＂＂；｝cout ＜＜ endl;｝｝｝；int main(）｛Graph g(5)；gaddEdge(0, 1)；gaddEdge(0, 2)；gaddEdge(1, 2)；gaddEdge(2, 3)；gaddEdge(3, 4)；gprintGraph(）；return 0;｝｀｀｀（三）深度优先遍历的实现｀｀｀cppinclude ＜iostream>include ＜vector>using namespace std;const int MAX_VERTEX_NUM ＝ 100;class Graph ｛private:int vertexNum;vector<int> adjListMAX_VERTEX_NUM;bool visitedMAX_VERTEX_NUM;public:Graph(int vNum) ｛vertexNum ＝ vNum;for （int i ＝ 0; i ＜ vertexNum; i+＋）｛visitedi ＝ false;｝｝void addEdge(int i, int j) ｛if （i ＞＝ 0 ＆＆ i ＜ vertexNum ＆＆ j ＞＝ 0 ＆＆ j ＜ vertexNum) ｛adjListipush_back(j)；adjListjpush_back(i)；｝｝void DFS(int v) ｛visitedv ＝ true;cout ＜＜ v ＜＜＂＂；for （int i ＝ 0; i ＜ adjListvsize(）； i+＋）｛int u ＝ adjListvi;if （！visitedu) ｛DFS(u)；｝｝｝void DFSTraversal(）｛for （int v ＝ 0; v ＜ vertexNum; v+＋）｛if （！visitedv) ｛DFS(v)；｝｝｝｝；int main(）｛Graph g(5)；gaddEdge(0, 1)；gaddEdge(0, 2)；gaddEdge(1, 2)；gaddEdge(2, 3)；gaddEdge(3, 4)；gDFSTraversal(）；return 0;｝｀｀｀（四）广度优先遍历的实现｀｀｀cppinclude ＜iostream>include ＜queue>include ＜vector>using namespace std;const int MAX_VERTEX_NUM ＝ 100; class Graph ｛private:int vertexNum;vector<int> adjListMAX_VERTEX_NUM; bool visitedMAX_VERTEX_NUM; public:Graph(int vNum) ｛vertexNum ＝ vNum;for （int i ＝ 0; i ＜ vertexNum; i+＋）｛visitedi ＝ false;｝｝void addEdge(int i, int j) ｛if （i ＞＝ 0 ＆＆ i ＜ vertexNum ＆＆ j ＞＝ 0 ＆＆ j ＜ vertexNum) ｛adjListipush_back(j)；adjListjpush_back(i)；｝｝void BFS(int v) ｛queue<int> q;visitedv ＝ true;qpush(v)；while （！qempty(））｛int u ＝ qfront(）；qpop(）；cout ＜＜ u ＜＜＂＂；for （int i ＝ 0; i ＜ adjListusize(）； i+＋）｛int w ＝ adjListui;if （！visitedw) ｛visitedw ＝ true;qpush(w)；｝｝｝｝void BFSTraversal(）｛for （int v ＝ 0; v ＜ vertexNum; v+＋）｛if （！visitedv) ｛BFS(v)；｝｝｝｝；int main(）｛Graph g(5)；gaddEdge(0, 1)；gaddEdge(0, 2)；gaddEdge(1, 2)；gaddEdge(2, 3)；gaddEdge(3, 4)；gBFSTraversal(）；return 0;｝｀｀｀（五）迪杰斯特拉算法的实现｀｀｀cppinclude ＜iostream>include ＜climits>include ＜vector>using namespace std;const int MAX_VERTEX_NUM ＝ 100; const int INFINITY ＝ INT_MAX; class Graph ｛private:int vertexNum;int adjMatrixMAX_VERTEX_NUMMAX_VERTEX_NUM;int distanceMAX_VERTEX_NUM;bool visitedMAX_VERTEX_NUM;public:Graph(int vNum) ｛vertexNum ＝ vNum;for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛adjMatrixij ＝ INFINITY;｝distancei ＝ INFINITY;visitedi ＝ false;｝｝void addEdge(int i, int j, int weight) ｛if （i ＞＝ 0 ＆＆ i ＜ vertexNum ＆＆ j ＞＝ 0 ＆＆ j ＜ vertexNum) ｛adjMatrixij ＝ weight;adjMatrixji ＝ weight;｝｝int minDistance(）｛int min ＝ INFINITY;int minIndex ＝－1;for （int v ＝ 0; v ＜ vertexNum; v+＋）｛if （！visitedv ＆＆ distancev ＜＝ min) ｛min ＝ distancev;minIndex ＝ v;｝｝return minIndex;｝void dijkstra(int src) ｛distancesrc ＝ 0;for （int count ＝ 0; count ＜ vertexNum 1; count+＋）｛int u ＝ minDistance(）；visitedu ＝ true;for （int v ＝ 0; v ＜ vertexNum; v+＋）｛if （！visitedv ＆＆ adjMatrixuv!＝ INFINITY ＆＆ distanceu!＝INFINITY ＆＆ distanceu ＋ adjMatrixuv ＜ distancev) ｛distancev ＝ distanceu ＋ adjMatrixuv;｝｝｝for （int i ＝ 0; i ＜ vertexNum; i+＋）｛cout ＜＜＂源点＂＜＜ src ＜＜＂到顶点＂＜＜ i ＜＜＂的最短距离为: ＂＜＜ distancei ＜＜ endl;｝｝｝；int main(）｛Graph g(5)；gaddEdge(0, 1, 2)；gaddEdge(0, 2, 4)；gaddEdge(1, 2, 1)；gaddEdge(1, 3, 7)；gaddEdge(2, 3, 3)；gaddEdge(3, 4, 5)；gdijkstra(0)；return 0;｝｀｀｀（六）弗洛伊德算法的实现｀｀｀cppinclude ＜iostream>include ＜climits>using namespace std;const int MAX_VERTEX_NUM ＝ 100; const int INFINITY ＝ INT_MAX; class Graph ｛private:int vertexNum;int adjMatrixMAX_VERTEX_NUMMAX_VERTEX_NUM;int distanceMAX_VERTEX_NUMMAX_VERTEX_NUM;public:Graph(int vNum) ｛vertexNum ＝ vNum;for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛adjMatrixij ＝ INFINITY;｝｝｝void addEdge(int i, int j, int weight) ｛if （i ＞＝ 0 ＆＆ i ＜ vertexNum ＆＆ j ＞＝ 0 ＆＆ j ＜ vertexNum) ｛adjMatrixij ＝ weight;｝｝void floyd(）｛for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛distanceij ＝ adjMatrixij;｝｝for （int k ＝ 0; k ＜ vertexNum; k+＋）｛for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛if （distanceik!＝ INFINITY ＆＆ distancekj!＝ INFINITY ＆＆distanceik ＋ distancekj ＜ distanceij) ｛distanceij ＝ distanceik ＋ distancekj;｝｝｝｝for （int i ＝ 0; i ＜ vertexNum; i+＋）｛for （int j ＝ 0; j ＜ vertexNum; j+＋）｛if （distanceij ＝＝ INFINITY) ｛cout ＜＜＂顶点＂＜＜ i ＜＜＂到顶点＂＜＜ j ＜＜＂的距离为: 无穷大" ＜＜ endl;｝ else ｛cout ＜＜＂顶点＂＜＜ i ＜＜＂到顶点＂＜＜ j ＜＜＂的距离为: ＂＜＜ distanceij ＜＜ endl;｝｝｝｝｝；int main(）｛Graph g(4)；gaddEdge(0, 1, 5)；gaddEdge(0, 3, 10)；gaddEdge(1, 2, 3)；gaddEdge(2, 3, 1)；gfloyd(）；return 0;｝｀｀｀五、实验结果分析（一）邻接矩阵和邻接表的比较邻接矩阵的优点是可以快速判断两个顶点之间是否有边相连，时间复杂度为O(1)。

数据结构实验报告图实验

数据结构实验———图实验报告

数据结构实验报告-答案.doc

数据结构实验报告-队列的操作

数据结构试验报告-图的基本操作

数据结构实验报告--图

数据结构实验报告

《数据结构》实验1实验报告

数据结构实验报告—图

数据结构--图的实验报告

数据结构实验报告及心得体会

数据结构试验报告一海龟作图（样例5）

数据结构图实验报告

数据结构实验报告

数据结构实验报告图的存储

数据结构实验报告(实验)

数据结构实验报告--图

数据结构实验报告 图

数据结构实验报告图