Chapter 10.1 Limit and Continuity of multivariat
Chapter 1 Limits and__ Continuity

-1 -2
Definition 2.7 Let f be a function defined on an open interval of the form (c, a) for some real number c, and let L be a real number. We say that the left-hand limit of f(x) as x approaches a from the left is L, and write lim f ( x ) = L, lim+ f ( x) = L,
lim f ( x ) = 3 2 ,
2 1 -2 -1 1 2
and say that the limit of f(x), as x approaches 1, is 3/2, or that f(x) approaches 3/2 as x approaches 1. Figure 6
6
x →1
x→a
if for every number ε>0, there is a number δ>0 such that
if 0 < x a < δ , then f ( x ) L < ε . y y=L+ε L y=L-ε o a-δ a a-δ x Figure 5
5
In order to understand the precise meaning of a function in Definition 2.5, let us begin to consider the behavior of a function 0.5 x + 1, x ≠ 1 f ( x) = x =1 0, as x approaches 1. From the graph of f shown in Figure 6, we can intuitively see that as x gets closer to 1 from both sides but x≠1, f(x) gets closer to 3/2. In this case, we use the notation
Section21LimitsandContinuity极限与连续

Section 2.1 Limits and Continuity 極限與連續【Topic 1. 極限(Limit)】1. 表示法x → c (念法:x 逼近c ) 意指 x 值任意靠近c ,但絕不等於c 值。
2. 對一個函數 f (x ) 而言,若x 逼近c (不等於c ),造成 f (x ) 逼近或等於某個特定L 值,則稱L 為函數 f 在 c 點的極限(值),可寫成 L x f cx =→)(lim 。
數學家柯西 (Cauchy) 提出極限的定義,稱為極限的δε−定義(δε− definition of a limit :較適理工學院研讀)。
3. x → c 當x 逼近c 有兩個方向:(1)當 x < c ,則 −→=−L x f cx )(lim ,稱為左極限;(1)當 x > c ,則 +→=+L x f cx )(lim ,稱為右極限。
4. 若極限存在,則 極限 L = 左極限 −L = 右極限 +L 。
(極限的存在性)5. 何時可以直接將x = c 代入函數 f (x ) 即可求得極限 )(lim x f cx →?請參考下列極限規則。
(稍後你會學到,當函數在 x = c 連續時,則極限等於函數值。
)6. 無窮極限與垂直漸近線: 無窮大 ∞ 與負無窮大 – ∞ 本身不是一個數值。
x → ∞ 代表 x 可以無止境的增大;x → – ∞ 代表 x 可以無止境的變小。
∞=→)(lim x f cx 代表當x 逐漸逼近c 時,f (x ) 可以無止境的增大;−∞=→)(lim x f cx 代表當x 逐漸逼近c 時,f (x ) 可以無止境的變小。
當 x 從 c 的左方或右方逼近 c 時,如果 f (x ) 趨近無窮大 (或負無窮大) [即單邊極限即可] ,我們就稱直線 x = c 是 f 函數圖形的一條垂直漸近線。
7. 在無窮遠處的極限與水平漸近線:L x f x =−∞→)(lim 或 L x f x =∞→)(lim 代表當x 在無窮遠處的極限值為 L 。
《微积分教学资料》calculusi(1)

A
x2 1 lim 2 x 1 x 1
x
1
3
x2 1 f ( x ) x 1 , x 1 x 1 3 ,
2
y
A
x 1 lim 2 x 1 x 1
2
1
x
The limit value does not depend on
how the function is defined at X0
x a
such that
f ( x) L
if
0 xa
then
Geometric interpretation of limit
f ( x)
L
L
L a a a
(4 x 5) 7 Example Prove that lim x 3
Proof 1.Guessing a value for . Let be a given positive number.
Sinx/x
0.84147098 0.95885108 0.97354586 0.98506736 0.99334665 0.99833417 0.99998339 0.99998333 0.99999583 0.99999983
sin x lim 1 x0 x
Caution: Notice the phrase “but x≠a” in the definition of limit. This means that in finding the limit of f(x) as x approaches a, we never consider x=a. In fact, f(x) need not even be defined when x=a. the only thing that matters is how f is defined near a.
Syllabus of Advanced Mathematics(II)

Syllabus of Advanced Mathematics(II)Code: Advanced Mathematics:130701X2Credits: 5Credit hours: 80Prerequisites: Elementary MathematicsSuitable For:Engineering Students一、课程简介本门课程是我校工科类各专业学生必修的一门重要的基础课,也是培养学生理性思维和创造能力的重要载体。
课程系统地介绍了多元函数微积分与无穷级数的基本概念、必要的基础理论和常用的运算方法。
其内容主要包括:多元函数微分学、曲线积分、曲面积分、无穷级数等。
Course DescriptionAs an important compulsory basic course of various kinds of engineering students in our university, Calculus I is to develop students’ ability of ratio nal thought and innovation. It provides the basic concepts of multi-variable calculus and power series and necessary theoretical foundation and common computational methods. This course consists of differential of multi-variable functions, multiple integrals, curve integrals, surface integrals and power series.二、课程目标通过本门课程的学习,要使学生掌握微积分的基本理论和基本方法,为学习后续课程和进一步获取数学知识奠定基础。
曼昆经济学原理英文版文案加习题答案10章

曼昆经济学原理英文版文案加习题答案10章EXTERNALITIESWHAT’S NEW IN THE S EVENTH EDITION:There is a new In the News feature on ―What Should We Do about Climate Change.‖LEARNING OBJECTIVES:By the end of this chapter, students should understand:what an externality is.why externalities can make market outcomes inefficient.the various government policies aimed at solving the problem of externalities.how people can sometimes solve the problem of externalities on their own.why private solutions to externalities sometimes do not work.CONTEXT AND PURPOSE:Chapter 10 is the first chapter in the microeconomic section of the text. It is the first chapter in a three-chapter sequence on the economics of the public sector. Chapter 10 addresses externalities—the uncompensated impact of one person’s actions on the well-being of a bystander. Chapter 11 will address public goods and common resources (goods that will be defined in Chapter 11) and Chapter 12 will address the tax system.In Chapter 10, different sources of externalities and a varietyof potential cures for externalities are addressed. Markets maximize total surplus to buyers and sellers in a market. However, if a market generates an externality (a cost or benefit to someone external to the market) the market equilibrium may not maximize the total benefit to society. Thus, in Chapter 10 we will see that while markets are usually a good way to organize economic activity, governments can sometimes improve market outcomes.182Chapter 10/Externalities ? 183KEY POINTS:When a transaction between a buyer and seller directly affects a third party, the effect is called an externality. If an activity yields negative externalities, such as pollution, the socially optimal quantity in a market is less than the equilibrium quantity. If an activity yields positive externalities, such as technology spillovers, the socially optimal quantity is greater than the equilibrium quantity.Governments pursue various policies to remedy the inefficiencies caused by externalities. Sometimes the government prevents socially inefficient activity by regulating behavior. Other times it internalizes an externality using corrective taxes. Another public policy is to issue permits. For example, the government could protect the environment by issuing a limited number of pollution permits. The result of this policy is largely the same as imposing corrective taxes on polluters.Those affected by externalities can sometimes solve the problem privately. For instance, when one business imposes anexternality on another business, the two businesses can internalize theexternality by merging. Alternatively, the interested parties can solve the problem by negotiating a contract. According to the Coase theorem, if people can bargain without cost, then they can always reach an agreement in which resources are allocated efficiently. In many cases, however, reaching a bargain among the many interested parties is difficult, so the Coase theorem does not apply.CHAPTER OUTLINE:I. Definition of externality : the uncompensated impact of one person’s actions on the well -being of a bystander.A. If the impact on the bystander is adverse, we say that there is a negative externality.B. If the impact on the bystander is beneficial, we say that there is a positive externality.C. In either situation, decisionmakers fail to take account of the external effects of their behavior.II. Externalities and Market InefficiencyA. Welfare Economics: A Recap1. The demand curve for a good reflects the value of that good to consumers, measured by theprice that the marginal buyer is willing to pay.2. The supply curve for a good reflects the cost of producing that good.3. In a free market, the price of a good brings supply and demand into balance in a way thatmaximizes total surplus (the difference between theconsumers’ valuation of the good and the sellers’ cost of producing it).184 ? Chapter 10/ExternalitiesB. Negative Externalities1. Example: An aluminum firm emits pollution during production.2. Social cost is equal to the private cost to the firm of producing the aluminum plus theexternal costs to those bystanders affected by the pollution. Thus, social cost exceeds the private cost paid by producers.3. The optimal amount of aluminum in the market will occur where total surplus is maximized.a. Total surplus is equal to the value of aluminum to consumers minus the cost (social cost)of producing it.b. This will occur where the social-cost curve intersects with demand curve. At this point,producing one more unit would lower total surplus because the value to consumers is less than the cost to produce it.4. Because the supply curve does not reflect the true cost of producing aluminum, the marketwill produce more aluminum than is optimal. 5. This negative externality could be internalized by a tax on producers for each unit ofaluminum sold.ALTERNATIVE CLASSROOM EXAMPLE:A coal-fired power plant emits pollution during production.Chapter 10/Externalities ? 1856. Definition of internalizing an externality: altering incentives so that people takeaccount of the external effects of their actions.7. In the News: The Externalities of Country Livinga. In The Lorax by Dr. Seuss, urbanization is criticized while country living is consideredmore environmentally friendly.b. This article from The New York Times describes research that suggests that city livingmay in fact be ―green er‖ because of the use of public transportation.C. Positive Externalities1. Example: education.2. Education yields positive externalities because better-educated voters lead to a bettergovernment. Crime rates also drop as the education level of the population rises.3. In this case, the demand curve does not reflect the social value of a good.4. If there is a positive externality, the social value of the good is greater than the private value,and the optimum quantity will be greater than the quantity produced in the market.5. To internalize a positive externality, the government could use a subsidy.Figure 3ALTERNATIVE CLASSROOM EXAMPLE:The purchase of a fire extinguisher when an individual lives in an apartment complex This is a good time to discuss why the government taxes goods like alcohol, tobacco, and gasoline. You will find that students have heard the phrase ―sin tax,‖ but they often do not understand why economists might support such taxes (given thedeadweight loss from taxes discussed in Chapter 8).186 ?Chapter 10/Externalities6. Case Study: Technology Spillovers, Industrial Policy, and Patent Protectiona. A technology spillover occurs when one firm’s research and production efforts impactanother firm’s access to technological advance.b. It is difficult to measure the amounts of technology spillover that occur and this leads toa debate over whether or not the government should pursue policies to encourage theproduction of technology.c. Patent protection is a type of technology policy of the government because it protectsthe rights of inventors who create new technologies. Without patents, there would beless incentive to develop new ideas and technologies.III. Public Policies toward ExternalitiesA. When an externality causes a market to reach an inefficient allocation of resources, thegovernment can respond in two ways.1. Command-and-control policies regulate behavior directly.2. Market-based policies provide incentives so that private decisionmakers will choose to solvethe problem on their own.B. Command-and-Control Policies: Regulation1. Externalities can be corrected by requiring or forbidding certain behaviors.2. In the United States, the Environmental Protection Agency (EPA) develops and enforcesregulations aimed at protecting the environment.3. EPA regulations include maximum levels of pollution allowed or required adoption of aparticular technology to reduce emissions.C. Market-Based Policy 1: Corrective Taxes and Subsidies1. Externalities can be internalized through the use of taxes and subsidies.2. Definition of corrective tax: a tax designed to induce private decisionmakers to takeaccount of the social costs that arise from a negative externality.a. These taxes are preferred by economists over regulation, because firms that can reducepollution with the least cost are likely to do so (to avoid the tax) while firms thatencounter high costs when reducing pollution will simply pay the tax.b. Thus, this tax allows firms that face the highest cost of reducing pollution to continue topollute while encouraging less pollution over all.Chapter 10/Externalities ?187c. Unlike other taxes, corrective taxes do not cause a reduction in total surplus. In fact,they increase economic well-being by forcing decisionmakers to take into account thecost of all of the resources being used when making decisions.3. Case Study: Why Is Gasoline Taxed So Heavily?a. In the United States, almost half of what drivers pay for gasoline goes to gas taxes.b. This is to correct for three negative externalities associated with driving: congestion,accidents, and pollution.D. Market-Based Policy 2: Tradable Pollution Permits1. Example: EPA regulations restrict the amount of pollution that two firms can emit at 300 tonsof glop per year. Firm A wants to increase its amount of pollution. Firm B agrees to decrease its pollution by the same amount if Firm A pays it $5 million.2. Social welfare is increased if the EPA allows this situation. Total pollution remains the sameso there are no external effects. If both firms are doing this willingly, it must make thembetter off.3. If the EPA issued permits to pollute and then allowed firms to sell them, this would alsoincrease social welfare. Firms that could control pollution most inexpensively would do so and sell their permits, while those who encounter high costs when reducing pollution would buyadditional permits.4. Tradable pollution permits and corrective taxes are similar in effect. In both cases, firms mustpay for the right to pollute.a. In the case of the tax, the government basically sets the price of pollution and firms thenchoose the level of pollution (given the tax) that maximizes their profit.b. If tradable pollution permits are used, the government chooses the level of pollution (intotal, for all firms) and firms then decide what they are willing to pay for these permits.188 ?Chapter 10/ExternalitiesE. Objections to the Economic Analysis of Pollution1. Some individuals dislike the idea of allowing companies to purchase the right to pollute.2. Economists point out that ―people face trade-offs‖ (Principle #1) and we must decide howmuch we would be willing to give up in exchange for no pollution. It would likely not beenough.3. A clean environment can be viewed as any other good that obeys the law of demand. Thelower the price of environmental protection, the more the public will want.F. In the News: What Should We Do about Climate Change?1. Many policy analysts believe that taxing carbon is the best approach to dealing with globalclimate change..2. This article from The New York Times explains how the revenue-neutral carbon tax works inBritish Columbia and argues for its implementation in the United States..IV. Private Solutions to ExternalitiesA. We do not necessarily need government involvement to correct externalities.B. The Types of Private Solutions1. Problems of externalities can sometimes be solved by moral codes and social sanctions.a. Do not litter.b. The Golden Rule2. Many charities have been established that deal with externalities. The governmentencourages this private solution by allowing a deduction for charitable contributions in thedetermination of taxable income.a. Sierra Club (environment)b. University Alumni Association (scholarships)3. The parties involved in this externality (either the seller and the bystander or the consumerand the bystander) can possibly enter into an agreement to correct the externality.C. The Coase Theorem1. Definition of Coase theorem: the proposition that if private parties can bargainwithout cost over the allocation of resources, they can solve the problem ofexternalities on their own.Chapter 10/Externalities ?1892. Example: Dick owns a dog Spot who disturbs a neighbor (Jane) with its barking.a. One possible solution to this problem would be for Jane to pay Dick to get rid of the dog.The amount that she would be willing to pay would be equal to her valuation of the costsof the barking. Dick would only agree to this if Jane paid him an amount greater than thevalue he places on owning Spot.b. Even if Jane could legally force Dick to get rid of Spot, another solution could occur. Dickcould pay Jane to let him keep the dog.3. Whatever the initial distribution of rights, the parties involved in an externality can potentiallysolve the problem themselves and reach an efficient outcome where both parties are betteroff.D. Why Private Solutions Do Not Always Work1. Definition of transaction costs: the costs that parties incur in the process ofagreeing and following through on a bargain.2. Coordination of all of the interested parties may be difficult so that bargaining breaks down.This is especially true when the number of interested parties is large. SOLUTIONS TO TEXT PROBLEMS:Quick Quizzes1. Examples of negative externalities include pollution, barking dogs, and consumption ofalcoholic beverages. Examples of positive externalitiesinclude the restoration of historicbuildings, research into new technologies, and education. (Many other examples of negativeand positive externalities are possible.) Market outcomes are inefficient in the presence ofexternalities because markets produce a larger quantity than is socially desirable when thereis a negative externality and a smaller quantity than is socially desirable when there is apositive externality. The market outcomes do not account for all of the costs (negativeexternalities) or benefits (positive externalities) to society.2. The town government might respond to the externality from the smoke in three ways: (1)regulation; (2) corrective taxes; or (3) tradable pollution permits.Regulation prohibiting pollution beyond some level is good because it is often effective atreducing pollution. But doing so successfully requires the government to have a lot ofinformation about the industries and the alternative technologies that those industries couldadopt.Corrective taxes are a useful way to reduce pollution because the tax can be increased to getpollution to a lower level and because the taxes raise revenue for the government. The tax ismore efficient than regulation because it gives factories economic incentives to reducepollution and to adopt new technologies that pollute less.The disadvantage of correctivetaxes is that the government needs to know a lot of information to pick the right tax rate.Tradable pollution permits are similar to corrective taxes but allow the firms to trade the rightto pollute with each other. As a result, the government does not need as much informationabout the firms’ technologies. The government c an simply set a limit on the total amo unt of190 ?Chapter 10/Externalitiespollution, issue permits for that amount, and allow the firms to trade the permits. Thisreduces pollution while allowing economic efficiency.3. Examples of private solutions to externalities include moral codes and social sanctions,charities, and relying on the interested parties entering into contracts with one other.The Coase theorem is the proposition that if private parties can bargain without cost over theallocation of resources, they can solve the problem of externalities on their own.Private economic participants are sometimes unable to solve the problems caused by anexternality because of transaction costs or because bargaining breaks down. This is mostlikely when the number of interested parties is large.Questions for Review1. Examples of negative externalities include pollution, barking dogs, and consumption ofalcoholic beverages. Examples of positive externalitiesinclude the restoration of historicbuildings, research into new technologies, and education. (Many other examples of negativeand positive externalities are possible.)2. Figure 1 illustrates the effect of a negative externality. The equilibrium quantity provided bythe market is Q market. Because of the externality, the social cost of production is greater thanthe private cost of production, so the social-cost curve is above the supply curve. The optimalquantity for society is Q optimum. The private market produces too much of the good becauseQ market is greater than Q optimum.Figure 13. The patent system helps society solve the externality problem from technology spillovers. Bygiving inventors exclusive use of their inventions for a certain period, the inventor cancapture much of the economic benefit of the invention. In doing so, the patent systemencourages research and technological advance, which benefits society through spillovereffects.Chapter 10/Externalities ?1914. Corrective taxes are taxes enacted to correct the effects ofa negative externality. Economistsprefer corrective taxes over regulations as a way to protect the environment from pollutionbecause they can reduce pollution at a lower cost to society.A tax can be set to reducepollution to the same level as a regulation. The tax has the advantage of letting the marketdetermine the least expensive way to reduce pollution. The tax gives firms incentives todevelop cleaner technologies to reduce the taxes they have to pay.5. Externalities can be solved without government intervention through moral codes and socialsanctions, charities, merging firms whose externalities affect each other, or by contract.6. According to the Coase theorem, you and your roommate will bargain over whether yourroommate will smoke in the room. If you value clean air morethan your roommate valuessmoking, the bargaining process will lead to your roommate not smoking. But if yourroommate values smoking more than you value clean air, the bargaining process will lead toyour roommate smoking. The outcome is efficient as long as transaction costs do not preventan agreement from taking place. The solution may be reached by one of you paying off theother either not to smoke or for the right to smoke.Quick Check Multiple Choice1. c2. b3. a4. c5. b6. cProblems and Applications1. The Club conveys a negative externality on other car owners because car thieves will notattempt to steal a car with The Club visibly in place. This means that they will move on toanother car. The Lojack system conveys a positive externality because thieves do not knowwhich cars have this technology. Therefore, they are less likely to steal any car. Policyimplications include a subsidy for car owners that use the Lojack technology or a tax onthose who use The Club.2. a. Fire extinguishers exhibit positive externalities becauseeven though people buy them fortheir own use, they may prevent fire from damaging the property of others.192 ? Chapter 10/ExternalitiesFigure 2b. Figure 2 illustrates the positive externality from fire extinguishers. Notice that the social-value curve is above the demand curve and the social-cost curve is the same as the supply curve.c. The market equilibrium level of output is denoted Q market and the efficient level of outputis denoted Q optimum . The quantities differ because in deciding to buy fire extinguishers, people don't account for the benefits they provide to others.d. A government policy that would result in the efficient outcome would be to subsidizepeople $10 for every fire extinguisher they buy. This would shift the demand curve up to the social-value curve, and the market quantity would increase to the optimum quantity. 3. a. The extra traffic is a negative externality because the social cost is greater than theprivate cost..b. Figure 3 shows the market for theater tickets. Because there is no external benefit, thesocial-value curve is the same as the demand curve in this case. However, the social-cost curve lies $5 above the supply curve at each quantity. The efficient level of output occurs where the social-value curve (which is demand in this case) and the social-cost curve intersect..Figure 3optimum market Price of TicketsChapter 10/Externalities ?193c. This is a positive externality because the social value of theater tickets is greater than theprivate value in this case.d. Figure 4 shows both the positive and the negative externalities.Figure 4e. A tax of $3 per ticket will lead to the efficient outcome. The market equilibrium quantitywill be equal to the social optimum.4. a. The market for alcohol is shown in Figure5. The social-value curve is the same as thedemand curve in this case. The social-cost curve is above the supply curve because ofthe negative externality from increased motor vehicle accidents caused by those whodrink and drive. The market equilibrium level of output is Q market and the efficient level of output is Q optimum.b. The triangular area between points A, B, and C represents the deadweight loss of themarket equilibrium. This area shows the amount by which social costs exceed socialvalue for the quantity of alcohol consumption beyond the efficient level.Figure 5194 ?Chapter 10/Externalities5. a. It is efficient to have different amounts of pollution reduction at different firms becausethe costs of reducing pollution differ across firms. If all firms were made to reducepollution by the same amount, the costs would be low at some firms and prohibitivelyhigh at others, imposing a greater burden overall.b. Command-and-control approaches that rely on uniformpollution reduction among firmsgive the firms no incentive to reduce pollution beyond the mandated amount. Instead,every firm will reduce pollution by just the amount required and no more.c. Corrective taxes or tradable pollution rights give firms greater incentives to reducepollution. Firms are rewarded by paying lower taxes or spending less on permits if theyfind methods to reduce pollution, so they have the incentive to engage in research onpollution control. The government does not have to figure out which firms can reducepollution the most?it lets the market give firms the incentive to reduce pollution on theirown.6. a. A t a price of $1.50, each Whovillian will consume 4 bottles of Zlurp. Eac h consumer’stotal willingness to pay is $14 (= $5 + $4 + $3 + $2). The total spent by each Whovillianon Zlurp is $6 (= $1.50 ? 4). Therefore, each consumer receives $8 in consumer surplus(=$14 ? $6).b. Total surplus would fall by $4 to $4.c. If Cindy Lou only consumes 3 bottles of Zlurp, her consumer surplus is $4.50. Herwillingness to pay for 3 bottles is $5 + $4 + $3 = $12. She pays $1.50 x 3 = $4.50 andthe externality is $1 x 3 = $3. Thus, Cindy Lou's consumer surplus is $12 - $4.50 - $3.00= $4.50. Cindy’s decision increases consumer surplus in Whoville by $0.50 ($4.50-$4.00).d. The $1 tax raises the price of a bottle of Zlurp to $2.50. (The entire tax will be borne byconsumers because supply is perfectly elastic.) Each resident will purchase only 3 bottlesat the higher price and each consumer’s total willingness to pay is now $12 (= $5 + $4 +$3). Each resident pays $7.50 (= $2.50 ? 3). Therefore, each resident receives $4.50($12-$7.50) in consumer surplus.Because each bottle has an external cost of $1, the per-resident external cost is $3 ($1per bottle x 3 bottles). The government collects $3 per resident in revenue. Total surpluswith the tax is equal to $4.50 - $3.00 + $3.00 = $4.50.e. Yes, because total surplus is now higher than before the tax.7. a. The externality is noise pollution. Ringo’s consumption of rock and roll music affectsLuciano, but Ringo does not consider that in deciding how loudly he plays his music.b. The landlord could impose a rule that music could not be played above a certain decibellevel. This could be inefficient because there would be no harm done by Ringo playinghis music loud if Luciano is not home.c. Ringo and Luciano could negotiate an agreement that might, for example, allow Ringo toplay his music loudly at certain times of the day. They mightnot be able to reach anagreement if the transaction costs are high or if bargaining fails because each holds outfor a better deal.Chapter 10/Externalities ?195 8. a. An improvement in the technology for controlling pollution would reduce the demand forpollution rights, shifting the demand curve to the left. Figure 6 illustrates what wouldhappen if there were a corrective tax, while Figure 7 shows the impact if there were afixed supply of pollution permits. In both figures, the curve labeled D1 is the originaldemand for pollution rights and the curve labeled D2 is the new demand for pollutionrights after the improvement in technology.Figure 6b. With a corrective tax, the price of pollution remains unchanged and the quantity ofpollution declines, as Figure 6 shows. With pollution permits, the price of pollutiondeclines and the quantity of pollution is unchanged, as Figure 7 illustrates.Figure 79. a. In terms of economic efficiency in the market for pollution, it does not matter if thegovernment distributes the permits or auctions them off, as long as firms can sell thepermits to each other. The only difference would be that the government could makemoney if it auctioned the permits off, thus allowing it to reduce taxes, which would help196 ?Chapter 10/Externalitiesreduce the deadweight loss from taxation. There could also be some deadweight lossoccurring if firms use resources to lobby for additional permits.b. If the government allocated the permits to firms who did not value them as highly asother firms, the firms could sell the permits to each other so they would end up in thehands of the firms who value them most highly. Thus, the allocation of permits amongfirms would not matter for efficiency. But it would affect the distribution of wealth,because those who got the permits and sold them would be better off.10. a. The firms with the highest cost of reducing pollution will buy permits rather than reducetheir pollution. Firms that can sell their permits for more than it costs them to reducetheir pollution will sell.Because firm B faces the highest costs of reducing pollution, $25 per unit, it will keep itsown 40 permits and buy 40 permits from the other firms, so that it can still pollute 80units. Thus, firm B does not reduce its pollution at all.Of the two remaining firms, firm A has the higher cost of reducing pollution so it willkeep its own 40 permits and reduce its pollution by 30 units at a cost of $20 x 30 units =$600.Firm C sells all 40 of its permits to firm B and reduces its pollution by 50 units at a cost of$10 × 50 = $500. The total cost of pollution reduction is。
Ergodic solenoidal homology

arXiv:math/0702501v1 [math.DG] 16 Feb 2007
˜ ´ VICENTE MUNOZ AND RICARDO PEREZ MARCO Abstract. We define generalized currents associated with immersions of abstract solenoids with a transversal measure. We realize geometrically the full real homology of a compact manifold with these generalized currents, and more precisely with immersions of minimal uniquely ergodic solenoids. This makes precise and geometric De Rham’s realization of the real homology by only using a restricted geometric subclass of currents. These generalized currents do extend Ruelle-Sullivan and Schwartzman currents. We extend Schwartzman theory beyond dimension 1 and provide a unified treatment of Ruelle-Sullivan and Schwartzman theories via Birkhoff’s ergodic theorem for the class of immersions of controlled solenoids. We develop some intersection theory of these new generalized currents that explains why the realization theorem cannot be achieved only with Ruelle-Sullivan currents.
《微积分英文》课件 (2)

Types of Limits
One-sided limits
Limits approached
from one direction
Limits at infinity
Behavior of functions at
infinity
● 02
第2章 Limits and Continuity
01 Definition of a limit
Explanation of what a limit is
02 Properties of limits
Key characteristics of limits
03 Calculating limits algebraically
Graphing functions by analyzing their derivatives and key points
Higher Order Derivatives
Second derivative
Rate of change of the rate of
change
nth derivative
● 03
第3章 Differentiation
Derivatives and Rates of
Change
A derivative is defined as the rate of change of a function at a given point. Notation for derivatives includes symbols such as f'(x) or dy/dx. Derivatives can be interpreted as rates of change in various realworld applications.
函数英文ppt课件ppt课件ppt课件

Definition: The composite function f (g (x)) is formed by replacing the input of function g with the output of function f. For example, if f (x)=x ^ 2 and g (x)=3x+2, then the composite function f (g (x))=(3x+2) ^ 2 for all x
The characteristics of linear functions include: when a>0, the function is an increasing function; When a<0, the function is a subtraction function.
Linear functions have a wide range of applications in real life, such as economics, statistics, and other fields.
Horizontal shift
Shifting a function Horizontally is equivalent to adding or subtracting a constant from all inputs For example, if f (x)=x ^ 2 is shifted left by 1 unit, it benefits (x-1) ^ 2
Exponential and logarithmic functions are also widely used in real life, such as in fields such as statistics and finance.
数学英语词汇(微积分)
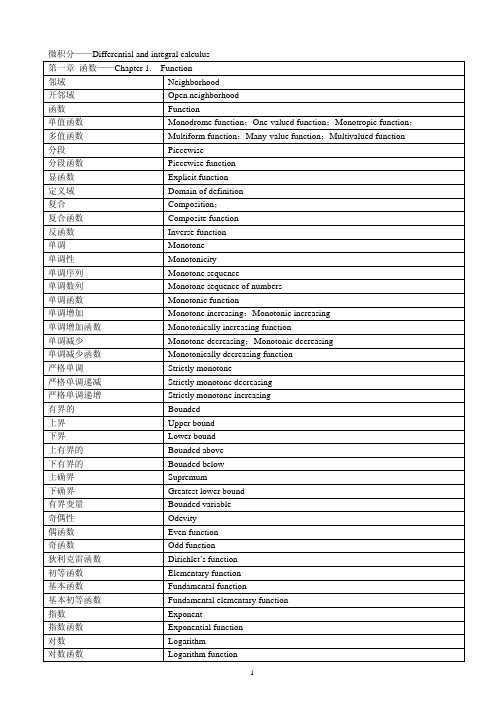
微积分——Differential and integral calculus第一章函数——Chapter 1. Function邻域 Neighborhoodneighborhood开邻域 Open函数 Functionfunction;One-valued function;Monotropic function;单值函数 Monodromefunction;Many-value function;Multivalued function多值函数 Multiform分段 Piecewisefunction分段函数 Piecewisefunction显函数 Explicit定义域Domain of definition复合 Composition;function复合函数 Composite反函数 Inversefunction单调 Monotone单调性 Monotonicity单调序列 Monotonesequence单调数列Monotone sequence of numbersfunction单调函数 Monotonicincreasing;Monotonic increasing单调增加 Monotone单调增加函数Monotonically increasing functiondecreasing;Monotonic decreasing单调减少 Monotone单调减少函数Monotonically decreasing functionmonotone严格单调 Strictly严格单调递减Strictly monotone decreasing严格单调递增Strictly monotone increasing有界的 Boundedbound上界 Upperbound下界 Lower上有界的 Boundedabovebelow下有界的 Bounded上确界 Supremumlowerbound下确界 Greatestvariable有界变量 Bounded奇偶性 Odevityfunction偶函数 Even奇函数 Oddfunctionfunction狄利克雷函数 Dirichlet’sfunction初等函数 Elementary基本函数 Fundamentalfunctionfunction基本初等函数 Fundamentalelementary指数 Exponentfunction指数函数 Exponential对数 Logarithmfunction对数函数 Logarithmfunctioncircular反三角函数 Inverse第二章极限与连续——Chapter 2. Limit and continuity序列 Sequencenumbersof数列 Sequence极限 Limit单侧 One-sided单侧极限 One-sidedlimit;Unilateral limitlimit左极限 Left收敛 Converge收敛性 Convergence发散 Diverge趋于零 Vanishingquantity无穷小量 Infinitelysmall无穷小的阶Order of infinitesimaldivisor零因子 Null无穷大量Infinitely large quantityinfinity正无穷大 Plusinfinity负无穷大 Minuslimit无穷极限 Infinite无穷大的阶Order of infinity单调收敛定理Monotone convergence theorem复利 Compoundinterest连续性 Continuity;Property of continuitycontinuous;Continuity from the left左连续 Leftcontinuous;Continuity from the right右连续 Rightpoint连续点 Continuitypoint不连续点 Discontinuitydiscontinuityof间断点 Point第一类不连续点Discontinuity point of the first kind第二类不连续点Discontinuity point of the second kinddiscontinuity可去间断点 Removablepoint;Moving singularitysingular可去奇点 Movablediscontinuity无穷间断点 Infinitediscontinuity跳跃间断点 Jumpvalue最大值 Greatesttheoremvalue介值定理 Intermediatepoint零点 Null第三章导数与微分——Chapter 3. Derivative and differential瞬时 Instantaneousspeed瞬时速度 Instantaneous导数 Derivativederivative单侧导数 One-sidedderivative;Derivative on the left左导数 Leftderivative;Derivative on the right;Progressive derivative右导数 Rightrule链式法则 Chain尖点 Cusp曲线的尖点Cusp of a curveorder高阶 Higherderivative;Higher derivative高阶导数 Higher-order微分 Differential可微的 Differentiablefunction不可微函数 Non-differentiableoperation微分运算 Differential求微分 Differentiatecoefficient微商 Differentialdifferential;Higher differential;Differentials of higher order 高阶微分 Higher-orderderivative相对导数 Relative弹性 Elasticity;Resilience第四章微分中值定理与导数应用——Chapter 4. differential mid-value theorem and application of derivative 中值 Mid-valuetheorem罗尔定理 Rolle’s未定式 Indeterminateformvalue;Extremal极值 Extreme极值点 Extremepointmaximum局部极大值 Localminimum局部极小值 Local凹 Concave凸 Convexpoint;knee拐点 Inflection渐近线 Asymptote;Asymptotic lineasymptote水平渐近线 Horizontalasymptote铅垂渐近线 Vertical第五章不定积分——Chapter 5. Indefinite integralfunction原函数 Primary积分 Integralintegral不定积分 Indefinite求积分 Quadrature可积的 Integrable曲线簇Family of curve积分法 Integration代换 Substitution换元积分法Integration by substitution分部积分法Integration by parts部分分式积分法Integration by partial fraction部分分式展开Partial fraction expansion第六章定积分——Chapter 6. Definite integralintegral定积分 Definite积分中值Integral mean value牛顿-莱布尼兹公式 Newton-Leibniz’sformulaofrotation旋转体 Bodyintegral广义积分 ImproperfunctionΓ函数 Gamma第七章级数——Chapter 7. Series级数 Seriesseries无穷级数 Infinite级数的项Term of a series部分和 Partialsumseries发散级数 Divergentseries振荡级数 Oscillatory几何级数 Geometricseries;Geometrical seriesseries正项级数 Positivetermseries调和级数 Harmoniccriterion达朗贝尔准则 D’Alembert’s绝对收敛 Absolutelyconvergent绝对收敛级数Absolutely convergent seriesconvergent条件收敛 Conditional条件收敛级数Conditional convergent series函数级数Series of functionsseries幂级数 Powerradius收敛半径 Convergencedomain;Region of convergence收敛域 Convergenceconvergence收敛区间 Intervalof逐项微分Term by term differentiationexpansion级数展开 Series幂级数展开Power series expansion;Expansion into power-seriesseries泰勒级数 Taylorformula泰勒公式 Taylor’sseries马克劳林级数 Maclaurin第八章多元函数微分学——Chapter 8. Differential for function of many variables 有界区域 Boundedregionregion开区域 Openregion连通区域 Connect不连通的 Disconnectedpoint;Interior point内点 Innerpoint外点 Outerpoint边界点 Frontiersurface柱面 Cylindrical母线 Generator柱的母线Element of cylinder;Generator of cylinder椭球 Ellipsoidcylinder椭圆柱面 Ellipticcylinder抛物柱面 Parabolic双曲面 Hyperboloid单叶双曲面Hyperboloid of one sheet双叶双曲面Hyperboloid of two sheets鞍点 Saddle齐次 Homogeneousfunction齐次函数 Homogeneouslimit;Two-limit二重极限 Double多重极限 Multiplelimitslimitn重极限 n-foldlimits累次极限 Repeatedderivative偏导数 Partialdifferential偏微分 Partialdifferential;Total differentiation全微分 Total高阶偏导数Higher-order partial derivative混合偏导数Mixed partial derivativecondition约束条件 Constraintequation拉格朗日方程 Lagrange拉格朗日乘数 Lagrangemultiplier第九章二重积分——Chapter 9. Double integral积分区域Domain of integrationelement;Differential of area面积元素 Areaintegral二重积分 Doubleintegral多重积分 Multipleintegral;Repeated integral累次积分 Iterated雅可比行列式 Jacobian广义二重积分 Improperintegraldouble第十章微分方程——Chapter 10. Differential equationequation微分方程 Differential常微分 Ordinarydifferentialequationdifferential常微分方程 Ordinary偏微分方程Partial differential equation微分方程的阶Order of a differential equation一阶微分方程Differential equation of first orderequationdifferential线性微分方程 Linearcondition初始条件 Initialvalue;Original value;Starting value初始值 Initialsolution特解 Particularequation齐次方程 Homogeneousequation 齐次微分方程 Homogeneousdifferential齐次线性微分方程Homogeneous linear differential equation非齐次的 Non-homogeneousequation非齐次微分方程 Non-homogeneousdifferential非齐次线性微分方程Non-homogeneous linear differential equation非线性的 Nonlinearequation非线性方程 Nonlinear常数变异法Method of variation of constantsequation;Differential equation of higher orderdifferential高阶微分方程 Higher-order高阶常微分方程Higher-order ordinary differential equation高阶偏微分方程Higher-order partial differential equationequation特征方程 Characteristicroot特征根 Characteristicequationintegral微分积分方程 Differention第十一章差分方程——Chapter 11. Difference equation差、差分 Differencedifference高阶差分 Higherequation差分方程 Difference。
Limits & Continuity

Limits and Continuity
Limits & Continuity
One-sided limits
The function f has the right-hand limit L as x approaches a from the right, written
xa
f a and f b , then there is at least one number c in a, b such that f c M .
Existence of Zeros of a Continuous Function
If f is a continuous function on a closed interval a, b , and if f a and f b have opposite signs, then there is at least one solution of the equation f x 0 in the interval
1.
f a is defined.
2.
xa
lim f x exists.
3.
xa
lim f x f a .
If any one of the above conditions is not satisfied, the function f is not continuous at x a . Then f is said to be discontinuous at x a . Also, f is continuous on an interval if f is continuous at every point in the interval.
大一高等数学a教材答案详解

大一高等数学a教材答案详解Chapter 1: Functions and Limits1.1 Introduction to FunctionsIn this chapter, we will explore the concept of functions and their properties. A function is a rule that assigns each element from one set to another set. It is represented by f(x), where x is an element from the domain and f(x) is the output value. Functions can be represented graphically, algebraically, or numerically.1.2 Limits and ContinuityLimits are used to describe the behavior of a function as x approaches a certain value. The limit of a function f(x) as x approaches a can be denoted as limₓ→a f(x). Continuity of a function is determined by the existence of a limit at a certain point and the value of the function at that point.1.3 DifferentiationDifferentiation is the process of finding the derivative of a function. The derivative represents the rate of change of a function at a particular point. It is denoted as f'(x) or dy/dx. The derivative can be used to find the slope of a tangent line, determine critical points, and analyze the behavior of functions.Chapter 2: Derivatives2.1 Basic Rules of DifferentiationIn this chapter, we will discuss the basic rules of differentiation. These rules include the power rule, product rule, quotient rule, and chain rule.These rules allow us to find the derivative of various functions by applying specific formulas and techniques.2.2 Applications of DerivativesDerivatives have various applications in real-life situations. They can be used to find maximum and minimum values, solve optimization problems, determine velocity and acceleration, and analyze growth and decay models. This chapter will address these applications and provide practical examples.2.3 Higher Order DerivativesHigher order derivatives refer to derivatives of derivatives. The second derivative represents the rate of change of the first derivative, while the third derivative represents the rate of change of the second derivative, and so on. Higher order derivatives can provide information about the curvature and concavity of a function.Chapter 3: Integration3.1 Antiderivatives and Indefinite IntegralsAntiderivatives are the opposite of derivatives. They represent the original function whose derivative is equal to a given function. The process of finding antiderivatives is called integration. The indefinite integral represents a family of functions, with the constant of integration accounting for the infinite number of antiderivatives.3.2 Definite IntegralsDefinite integrals are used to calculate the accumulated change of a function over a specific interval. The definite integral of a function f(x) froma tob is denoted as ∫[a, b] f(x) dx. It represents the area under the curve of the function between the limits a and b. This chapter will discuss the properties and techniques of definite integration.3.3 Applications of IntegrationIntegration has various applications, including calculating areas and volumes, solving differential equations, determining average values, and analyzing accumulation problems. These applications will be explored in this chapter, along with practical examples.Chapter 4: Techniques of Integration4.1 Integration by SubstitutionIntegration by substitution is a technique used to simplify integrals by replacing variables or functions. It involves choosing an appropriate substitution and applying the chain rule in reverse. This method can be used to solve complex integrals and make them more manageable.4.2 Integration by PartsIntegration by parts is another integration technique that allows us to find the integral of a product of two functions. It involves choosing one function to differentiate and the other function to integrate. This method is useful for integrating products of functions such as polynomials, exponentials, logarithms, and trigonometric functions.4.3 Trigonometric IntegralsTrigonometric integrals involve integrating functions that contain trigonometric functions like sine, cosine, tangent, secant, etc. These integralscan be solved using trigonometric identities and substitution techniques specific to trigonometric functions.In conclusion, the first-year high school mathematics A textbook provides a comprehensive introduction to functions, limits, derivatives, and integration. It covers the fundamental concepts and techniques necessary for further study in advanced mathematics. By understanding and applying the principles discussed in this textbook, students will acquire a solid foundation in calculus and its applications.。
高等数学(微积分学)专业术语名词概念定理等英汉对照

高等数学(微积分学)专业术语名词概念定理等英汉对照目录第一部分英汉微积分词汇Part 1 English-Chinese Calculus Vocabulary第一章函数与极限Chapter 1 function and Limi t (1)第二章导数与微分Chapter 2 Derivative and Differential (2)第三章微分中值定理Chapter 3 Mean Value theorem of differentials and the Application of Derivatives (3)第四章不定积分Chapter 4 Indefinite Intergrals (3)第五章定积分Chapter 5 Definite Integral (3)第六章定积分的应用Chapter 6 Application of the Definite Integrals (4)第七章空间解析几何与向量代数Chapter 7 Space Analytic Geomertry and Vector Algebra (4) 第八章多元函数微分法及其应用Chapter 8 Differentiation of functions Several variables and Its Application (5)第九章重积分Multiple Integrals (6)第十章曲线积分与曲面积分Chapter 10 Line(Curve ) Integrals and Surface Integral s (6) 第十一章无穷级数Chapter 11 Infinite Series (6)第十二章微分方程Chapter 12 Differential Equation (7)第二部分定理定义公式的英文表达Part 2 English Expression for Theorem,Definition and Formula第一章函数与极限Chapter 1 Function and Limi t (19)1.1映射与函数(Mapping and Function ) (19)1.2数列的极限(Limit of the Sequence of Number) (20)1.3函数的极限(Limit of Function) (21)1.4无穷小与无穷大(Infinitesimal and Inifinity) (23)1.5极限运算法则(Operation Rule of Limit) (24)1.6极限存在准则两个重要的极限(Rule for theExistence of Limits Two Important Limits) (25)1.7无穷小的比较(The Comparison of infinitesimal) (26)1.8函数的连续性与间断点(Continuity of FunctionAnd Discontinuity Points) (28)1.9连续函数的运酸与初等函数的连续性(OperationOf Continuous Functions and Continuity ofElementary Functions) (28)1.10闭区间上联系汗水的性质(Properties ofContinuous Functions on a Closed Interval) (30)第二章导数与数分Chapter2 Derivative and Differential (31)2.1 导数的概念(The Concept of Derivative) (31)2.2 函数的求导法则(Rules for Finding Derivatives) (33)2.3 高阶导数(Higher-order Derivatives) (34)2.4 隐函数及由参数方程所确定的函数的导数相关变化率(Derivatives ofImplicit Functions and Functions Determined by Parametric Equation andCorrelative Change Rate) (34)2.5 函数的微分(Differential of a Function) (35)第三章微分中值定理与导数的应用Chapter 3 Mean Value Theorem of Differentials and theApplication of Derivatives (36)3.1 微分中值定理(The Mean Value Theorem) (36)3.2 洛必达法则(L’Hopital’s Rule) (38)3.3 泰勒公式(Taylor’s Formula) (41)3.4 函数的单调性和曲线的凹凸性(Monotonicityof Functions and Concavity of Curves) (43)3.5 函数的极值与最大最小值(Extrema, Maximaand Minima of Functions) (46)3.6 函数图形的描绘(Graphing Functions) (49)3.7 曲率(Curvature) (50)3.8 方程的近似解(Solving Equation Numerically) (53)第四章不定积分Chapter 4Indefinite Integrals (54)4.1 不定积分的概念与性质(The Concept andProperties of Indefinite Integrals) (54)4.2 换元积分法(Substitution Rule for Indefinite Integrals) (56)4.3 分部积分法(Integration by Parts) (57)4.4 有理函数的积分(Integration of Rational Functions) (58)第五章定积分Chapter 5 Definite Integrals (61)5.1 定积分的概念和性质(Concept of Definite Integraland its Properties) (61)5.2 微积分基本定理(Fundamental Theorem of Calculus) (67)5.3 定积分的换元法和分部积分法(Integration by Substitution andDefinite Integrals by Parts) (69)5.4 反常积分(Improper Integrals) (70)第六章定积分的应用Chapter 6 Applications of the Definite Integrals (75)6.1 定积分的元素法(The Element Method of Definite Integra (75)6.2 定积分在几何学上的应用(Applications of the DefiniteIntegrals to Geometry) (76)6.3 定积分在物理学上的应用(Applications of the DefiniteIntegrals to Physics) (79)第七章空间解析几何与向量代数Chapter 7 Space Analytic Geometry and Vector Algebar (80)7.1 向量及其线性运算(Vector and Its Linear Operation) (80)7.2 数量积向量积(Dot Product and Cross Product) (86)7.3 曲面及其方程(Surface and Its Equation) (89)7.4 空间曲线及其方程(The Curve in Three-space and Its Equation (91)7.5 平面及其方程(Plane in Space and Its Equation) (93)7.6 空间直线及其方程(Lines in and Their Equations) (95)第八章多元函数微分法及其应用Chapter 8 Differentiation of Functions of SeveralVariables and Its Application (99)8.1 多元函数的基本概念(The Basic Concepts of Functionsof Several Variables) (99)8.2 偏导数(Partial Derivative) (102)8.3 全微分(Total Differential) (103)8.4 链式法则(The Chain Rule) (104)8.5 隐函数的求导公式(Derivative Formula for Implicit Functions). (104)8.6 多元函数微分学的几何应用(Geometric Applications of Differentiationof Ffunctions of Severalvariables) (106)8.7方向导数与梯度(Directional Derivatives and Gradients) (107)8.8多元函数的极值(Extreme Value of Functions of Several Variables) (108)第九章重积分Chapter 9 Multiple Integrals (111)9.1二重积分的概念与性质(The Concept of Double Integralsand Its Properities) (111)9.2二重积分的计算法(Evaluation of double Integrals) (114)9.3三重积分(Triple Integrals) (115)9.4重积分的应用(Applications of Multiple Itegrals) (120)第十章曲线积分与曲面积分Chapte 10 Line Integrals and Surface Integrals (121)10.1 对弧长的曲线积分(line Intergrals with Respect to Arc Length) (121)10.2 对坐标的曲线积分(Line Integrals with respect toCoordinate Variables) (123)10.3 格林公式及其应用(Green's Formula and Its Applications) (124)10.4 对面积的曲面积分(Surface Integrals with Respect to Aarea) (126)10.5 对坐标的曲面积分(Surface Integrals with Respect toCoordinate Variables) (128)10.6 高斯公式通量与散度(Gauss's Formula Flux and Divirgence) (130)10.7 斯托克斯公式环流量与旋度(Stokes's Formula Circulationand Rotation) (131)第十一章无穷级数Chapter 11 Infinite Series (133)11.1 常数项级数的概念与性质(The concept and Properties ofThe Constant series) (133)11.2 常数项级数的审敛法(Test for Convergence of the Constant Series) (137)11.3 幂级数(power Series). (143)11.4 函数展开成幂级数(Represent the Function as Power Series) (148)11.5 函数的幂级数展开式的应用(the Appliacation of the Power Seriesrepresentation of a Function) (148)11.6 函数项级数的一致收敛性及一致收敛级数的基本性质(The UnanimousConvergence of the Series of Functions and Its properties) (149)11.7 傅立叶级数(Fourier Series) (152)11.8 一般周期函数的傅立叶级数(Fourier Series of Periodic Functions) (153)第十二章微分方程Chapter 12 Differential Equation (155)12.1微分方程的基本概念(The Concept of DifferentialEquation) (155)12.2可分离变量的微分方程(Separable Differential Equation) (156)12.3齐次方程(Homogeneous Equation) (156)12.4 一次线性微分方程(Linear Differential Equation of theFirst Order) (157)12.5全微分方程(Total Differential Equation) (158)12.6可降阶的高阶微分方程(Higher-order DifferentialEquation Turned to Lower-order DifferentialEquation) (159)12.7高阶线性微分方程(Linear Differential Equation of HigherOrder) (159)12.8常系数齐次线性微分方程(Homogeneous LinearDifferential Equation with Constant Coefficient) (163)12.9常系数非齐次线性微分方程(Non HomogeneousDifferential Equation with Constant Coefficient) (164)12.10 欧拉方程(Euler Equation) (164)12.11 微分方程的幂级数解法(Power Series Solutionto Differential Equation) (164)第三部分常用数学符号的英文表达Part 3 English Expression of the Mathematical Symbol in Common Use第一部分英汉微积分词汇Part1 English-Chinese Calculus V ocabulary 第一章函数与极限Chapter1 Function and Limit集合set元素element子集subset空集empty set并集union交集intersection差集difference of set基本集basic set补集complement set直积direct product笛卡儿积Cartesian product开区间open interval闭区间closed interval半开区间half open interval有限区间finite interval区间的长度length of an interval无限区间infinite interval领域neighborhood领域的中心centre of a neighborhood领域的半径radius of a neighborhood左领域left neighborhood右领域right neighborhood 映射mappingX到Y的映射mapping of X ontoY 满射surjection单射injection一一映射one-to-one mapping双射bijection算子operator变化transformation函数function逆映射inverse mapping复合映射composite mapping自变量independent variable因变量dependent variable定义域domain函数值value of function函数关系function relation值域range自然定义域natural domain单值函数single valued function多值函数multiple valued function 单值分支one-valued branch函数图形graph of a function绝对值函数absolute value符号函数sigh function整数部分integral part阶梯曲线step curve当且仅当if and only if(iff)分段函数piecewise function上界upper bound下界lower bound有界boundedness无界unbounded函数的单调性monotonicity of a function 单调增加的increasing单调减少的decreasing单调函数monotone function函数的奇偶性parity(odevity) of a function对称symmetry偶函数even function奇函数odd function函数的周期性periodicity of a function周期period反函数inverse function直接函数direct function复合函数composite function中间变量intermediate variable函数的运算operation of function基本初等函数basic elementary function初等函数elementary function幂函数power function指数函数exponential function对数函数logarithmic function三角函数trigonometric function反三角函数inverse trigonometric function 常数函数constant function双曲函数hyperbolic function双曲正弦hyperbolic sine双曲余弦hyperbolic cosine双曲正切hyperbolic tangent反双曲正弦inverse hyperbolic sine反双曲余弦inverse hyperbolic cosine反双曲正切inverse hyperbolic tangent极限limit数列sequence of number收敛convergence收敛于 a converge to a发散divergent极限的唯一性uniqueness of limits收敛数列的有界性boundedness of a convergent sequence子列subsequence函数的极限limits of functions函数()f x当x趋于x0时的极限limit of functions () f x as x approaches x0左极限left limit右极限right limit单侧极限one-sided limits水平渐近线horizontal asymptote无穷小infinitesimal无穷大infinity铅直渐近线vertical asymptote夹逼准则squeeze rule单调数列monotonic sequence高阶无穷小infinitesimal of higher order低阶无穷小infinitesimal of lower order同阶无穷小infinitesimal of the same order 等阶无穷小equivalent infinitesimal函数的连续性continuity of a function增量increment函数()f x在x0连续the function ()f x is continuous at x0左连续left continuous右连续right continuous区间上的连续函数continuous function函数()f x在该区间上连续function ()f x is continuous on an interval不连续点discontinuity point第一类间断点discontinuity point of the first kind第二类间断点discontinuity point of the second kind初等函数的连续性continuity of the elementary functions定义区间defined interval最大值global maximum value (absolute maximum)最小值global minimum value (absolute minimum)零点定理the zero point theorem介值定理intermediate value theorem第二章导数与微分Chapter2 Derivative and Differential速度velocity匀速运动uniform motion平均速度average velocity瞬时速度instantaneous velocity圆的切线tangent line of a circle切线tangent line切线的斜率slope of the tangent line位置函数position function导数derivative可导derivable函数的变化率问题problem of the change rate of a function 导函数derived function左导数left-hand derivative右导数right-hand derivative单侧导数one-sided derivatives()f x在闭区间【a,b】上可导()f x is derivable on the closed interval [a,b]切线方程tangent equation角速度angular velocity成本函数cost function边际成本marginal cost链式法则chain rule隐函数implicit function显函数explicit function二阶函数second derivative三阶导数third derivative高阶导数nth derivative莱布尼茨公式Leibniz formula对数求导法log- derivative参数方程parametric equation相关变化率correlative change rata微分differential可微的differentiable函数的微分differential of function自变量的微分differential of independent variable微商differential quotient间接测量误差indirect measurement error 绝对误差absolute error 相对误差relative error第三章微分中值定理与导数的应用Chapter3 MeanValue Theorem of Differentials and the Application of Derivatives 罗马定理Rolle’s theorem费马引理Fermat’s lemma拉格朗日中值定理Lagrange’s mean value theorem驻点stationary point稳定点stable point临界点critical point辅助函数auxiliary function拉格朗日中值公式Lagrange’s mean value formula柯西中值定理Cauchy’s mean value theorem洛必达法则L’Hospital’s Rule0/0型不定式indeterminate form of type 0/0不定式indeterminate form泰勒中值定理Taylor’s mean value theorem泰勒公式Taylor formula余项remainder term拉格朗日余项Lagrange remainder term 麦克劳林公式Maclaurin’s formula佩亚诺公式Peano remainder term凹凸性concavity凹向上的concave upward, cancave up凹向下的,向上凸的concave downward’concave down拐点inflection point函数的极值extremum of function极大值local(relative) maximum最大值global(absolute) mximum极小值local(relative) minimum最小值global(absolute) minimum目标函数objective function曲率curvature弧微分arc differential平均曲率average curvature曲率园circle of curvature曲率中心center of curvature曲率半径radius of curvature渐屈线evolute渐伸线involute根的隔离isolation of root隔离区间isolation interval切线法tangent line method第四章不定积分Chapter4 Indefinite Integrals原函数primitive function(antiderivative) 积分号sign of integration被积函数integrand积分变量integral variable积分曲线integral curve积分表table of integrals换元积分法integration by substitution分部积分法integration by parts分部积分公式formula of integration by parts有理函数rational function真分式proper fraction假分式improper fraction第五章定积分Chapter5 Definite Integrals曲边梯形trapezoid with曲边curve edge窄矩形narrow rectangle曲边梯形的面积area of trapezoid with curved edge积分下限lower limit of integral积分上限upper limit of integral积分区间integral interval分割partition积分和integral sum可积integrable矩形法rectangle method积分中值定理mean value theorem of integrals函数在区间上的平均值average value of a function on an integvals牛顿-莱布尼茨公式Newton-Leibniz formula微积分基本公式fundamental formula of calculus换元公式formula for integration by substitution 递推公式recurrence formula反常积分improper integral反常积分发散the improper integral is divergent反常积分收敛the improper integral is convergent无穷限的反常积分improper integral on an infinite interval无界函数的反常积分improper integral of unbounded functions绝对收敛absolutely convergent第六章定积分的应用Chapter6 Applications of the Definite Integrals元素法the element method面积元素element of area平面图形的面积area of a luane figure直角坐标又称“笛卡儿坐标(Cartesian coordinates)”极坐标polar coordinates抛物线parabola椭圆ellipse旋转体的面积volume of a solid of rotation旋转椭球体ellipsoid of revolution, ellipsoid of rotation曲线的弧长arc length of acurve可求长的rectifiable光滑smooth功work水压力water pressure引力gravitation变力variable force第七章空间解析几何与向量代数Chapter7 Space Analytic Geometry and Vector Algebra向量vector自由向量free vector单位向量unit vector零向量zero vector相等equal平行parallel向量的线性运算linear poeration of vector 三角法则triangle rule平行四边形法则parallelogram rule交换律commutative law结合律associative law负向量negative vector差difference分配律distributive law空间直角坐标系space rectangular coordinates坐标面coordinate plane卦限octant向量的模modulus of vector向量a与b的夹角angle between vector a and b方向余弦direction cosine方向角direction angle向量在轴上的投影projection of a vector onto an axis数量积,外积,叉积scalar product,dot product,inner product 曲面方程equation for a surface球面sphere旋转曲面surface of revolution母线generating line轴axis圆锥面cone顶点vertex旋转单叶双曲面revolution hyperboloids of one sheet旋转双叶双曲面revolution hyperboloids of two sheets柱面cylindrical surface ,cylinder圆柱面cylindrical surface准线directrix抛物柱面parabolic cylinder二次曲面quadric surface椭圆锥面dlliptic cone椭球面ellipsoid单叶双曲面hyperboloid of one sheet双叶双曲面hyperboloid of two sheets旋转椭球面ellipsoid of revolution椭圆抛物面elliptic paraboloid旋转抛物面paraboloid of revolution双曲抛物面hyperbolic paraboloid马鞍面saddle surface 椭圆柱面elliptic cylinder双曲柱面hyperbolic cylinder抛物柱面parabolic cylinder空间曲线space curve空间曲线的一般方程general form equations of a space curve 空间曲线的参数方程parametric equations of a space curve螺转线spiral螺矩pitch投影柱面projecting cylinder投影projection平面的点法式方程pointnorm form eqyation of a plane法向量normal vector平面的一般方程general form equation of a plane两平面的夹角angle between two planes 点到平面的距离distance from a point to a plane空间直线的一般方程general equation of a line in space方向向量direction vector直线的点向式方程pointdirection form equations of a line方向数direction number直线的参数方程parametric equations of a line两直线的夹角angle between two lines垂直perpendicular直线与平面的夹角angle between a line and a planes平面束pencil of planes平面束的方程equation of a pencil of planes行列式determinant系数行列式coefficient determinant第八章多元函数微分法及其应用Chapter8 Differentiation of Functions of Several Variables and Its Application一元函数function of one variable多元函数function of several variables内点interior point外点exterior point边界点frontier point,boundary point聚点point of accumulation开集openset闭集closed set连通集connected set开区域open region闭区域closed region有界集bounded set无界集unbounded setn维空间n-dimentional space二重极限double limit多元函数的连续性continuity of function of seveal连续函数continuous function不连续点discontinuity point一致连续uniformly continuous偏导数partial derivative对自变量x的偏导数partial derivative with respect to independent variable x高阶偏导数partial derivative of higher order二阶偏导数second order partial derivative 混合偏导数hybrid partial derivative全微分total differential偏增量oartial increment偏微分partial differential全增量total increment可微分differentiable必要条件necessary condition充分条件sufficient condition叠加原理superpostition principle全导数total derivative中间变量intermediate variable隐函数存在定理theorem of the existence of implicit function 曲线的切向量tangent vector of a curve法平面normal plane向量方程vector equation向量值函数vector-valued function切平面tangent plane法线normal line方向导数directional derivative梯度gradient 数量场scalar field梯度场gradient field向量场vector field势场potential field引力场gravitational field引力势gravitational potential曲面在一点的切平面tangent plane to a surface at a point曲线在一点的法线normal line to a surface at a point无条件极值unconditional extreme values 条件极值conditional extreme values拉格朗日乘数法Lagrange multiplier method拉格朗日乘子Lagrange multiplier经验公式empirical formula最小二乘法method of least squares均方误差mean square error第九章重积分Chapter9 Multiple Integrals二重积分double integral可加性additivity累次积分iterated integral体积元素volume element三重积分triple integral直角坐标系中的体积元素volume element in rectangular coordinate system柱面坐标cylindrical coordinates柱面坐标系中的体积元素volume element in cylindrical coordinate system球面坐标spherical coordinates球面坐标系中的体积元素volume element in spherical coordinate system反常二重积分improper double integral曲面的面积area of a surface质心centre of mass静矩static moment密度density形心centroid转动惯量moment of inertia参变量parametric variable第十章曲线积分与曲面积分Chapter10 Line(Curve)Integrals and Surface Integrals对弧长的曲线积分line integrals with respect to arc hength第一类曲线积分line integrals of the first type对坐标的曲线积分line integrals with respect to x,y,and z第二类曲线积分line integrals of the second type有向曲线弧directed arc单连通区域simple connected region复连通区域complex connected region格林公式Green formula第一类曲面积分surface integrals of the first type对面的曲面积分surface integrals with respect to area有向曲面directed surface对坐标的曲面积分surface integrals with respect to coordinate elements第二类曲面积分surface integrals of the second type有向曲面元element of directed surface高斯公式gauss formula拉普拉斯算子Laplace operator格林第一公式Green’s first formula通量flux散度divergence斯托克斯公式Stokes formula环流量circulation旋度rotation,curl第十一章无穷级数Chapter11 Infinite Series一般项general term部分和partial sum余项remainder term等比级数geometric series几何级数geometric series公比common ratio调和级数harmonic series柯西收敛准则Cauchy convergence criteria, Cauchy criteria for convergence正项级数series of positive terms达朗贝尔判别法D’Alembert test柯西判别法Cauchy test 交错级数alternating series绝对收敛absolutely convergent条件收敛conditionally convergent柯西乘积Cauchy product函数项级数series of functions发散点point of divergence收敛点point of convergence收敛域convergence domain和函数sum function幂级数power series幂级数的系数coeffcients of power series 阿贝尔定理Abel Theorem收敛半径radius of convergence收敛区间interval of convergence泰勒级数Taylor series麦克劳林级数Maclaurin series二项展开式binomial expansion近似计算approximate calculation舍入误差round-off error,rounding error欧拉公式Euler’s formula魏尔斯特拉丝判别法Weierstrass test三角级数trigonometric series振幅amplitude角频率angular frequency初相initial phase矩形波square wave谐波分析harmonic analysis直流分量direct component基波fundamental wave二次谐波second harmonic三角函数系trigonometric function system 傅立叶系数Fourier coefficient傅立叶级数Forrier series周期延拓periodic prolongation正弦级数sine series余弦级数cosine series奇延拓odd prolongation偶延拓even prolongation傅立叶级数的复数形式complex form of Fourier series第十二章微分方程Chapter12 Differential Equation解微分方程solve a dirrerential equation 常微分方程ordinary differential equation偏微分方程partial differential equation,PDE微分方程的阶order of a differential equation微分方程的解solution of a differential equation微分方程的通解general solution of a differential equation初始条件initial condition微分方程的特解particular solution of a differential equation 初值问题initial value problem微分方程的积分曲线integral curve of a differential equation 可分离变量的微分方程variable separable differential equation 隐式解implicit solution隐式通解inplicit general solution衰变系数decay coefficient衰变decay齐次方程homogeneous equation一阶线性方程linear differential equation of first order非齐次non-homogeneous齐次线性方程homogeneous linear equation非齐次线性方程non-homogeneous linear equation常数变易法method of variation of constant暂态电流transient stata current稳态电流steady state current伯努利方程Bernoulli equation全微分方程total differential equation积分因子integrating factor高阶微分方程differential equation of higher order悬链线catenary高阶线性微分方程linera differential equation of higher order 自由振动的微分方程differential equation of free vibration强迫振动的微分方程differential equation of forced oscillation 串联电路的振荡方程oscillation equation of series circuit二阶线性微分方程second order linera differential equation线性相关linearly dependence线性无关linearly independce二阶常系数齐次线性微分方程second order homogeneour linear differential equation with constant coefficient二阶变系数齐次线性微分方程second order homogeneous linear differential equation with variable coefficient特征方程characteristic equation无阻尼自由振动的微分方程differential equation of free vibration with zero damping 固有频率natural frequency 简谐振动simple harmonic oscillation,simple harmonic vibration微分算子differential operator待定系数法method of undetermined coefficient共振现象resonance phenomenon欧拉方程Euler equation幂级数解法power series solution数值解法numerial solution勒让德方程Legendre equation微分方程组system of differential equations常系数线性微分方程组system of linera differential equations with constant coefficient第二部分定理定义公式的英文表达Part2 English Expression for Theorem, Definition and Formula第一章函数与极限Chapter 1 Function and Limit1.1 映射与函数 (Mapping and Function)一、集合 (Set)二、映射 (Mapping)映射概念 (The Concept of Mapping) 设X , Y 是两个非空集合 , 如果存在一个法则f ,使得对X 中每个元素x ,按法则f ,在Y 中有唯一确定的元素y 与之对应 , 则称f 为从X 到 Y 的映射 , 记作:f X Y →。
英文高等数学教材答案

英文高等数学教材答案Chapter 1: Functions and their Graphs1.1 Introduction to Functions1.1.1 Definition of a FunctionA function is a relation that assigns a unique output value to each input value. It can be represented symbolically as f(x) or y = f(x), where x is the input variable and y is the output variable.1.1.2 Notation and TerminologyIn function notation, f(x) represents the output value corresponding to the input value x. The domain of a function is the set of all possible input values, while the range is the set of all possible output values.1.2 Graphs of Functions1.2.1 Cartesian Coordinate SystemThe Cartesian coordinate system consists of two perpendicular number lines, the x-axis and the y-axis. The point of intersection is called the origin and is labeled (0, 0). The x-coordinate represents the horizontal distance from the origin, while the y-coordinate represents the vertical distance.1.2.2 Graphical Representation of FunctionsThe graph of a function is a visual representation that shows the relationship between the input and output values. It consists of all points (x, f(x)) where x is in the domain of the function. The shape of the graph depends on the nature of the function.1.3 Properties of Functions1.3.1 Even and Odd FunctionsAn even function is symmetric with respect to the y-axis, meaning f(-x) = f(x) for all x in the domain. An odd function is symmetric with respect to the origin, meaning f(-x) = -f(x) for all x in the domain.1.3.2 Increasing and Decreasing FunctionsA function is increasing if the output values increase as the input values increase. It is decreasing if the output values decrease as the input values increase. A function can also be constant if the output values remain the same for all inputs.Chapter 2: Limits and Continuity2.1 Introduction to Limits2.1.1 Limit of a FunctionThe limit of a function f(x) as x approaches a particular value c, denoted as lim[x→c] f(x), describes the behavior of the function near that point. It represents the value that the function approaches as x gets arbitrarily close to c.2.1.2 One-Sided LimitsOne-sided limits consider the behavior of the function from only one side of the point. The limit from the left, lim[x→c-] f(x), looks at the behavior as x approaches c from values less than c. The limit from the right, lim[x→c+] f(x), considers the behavior as x approaches c from values greater than c.2.2 Techniques for Evaluating Limits2.2.1 Direct SubstitutionDirect substitution involves substituting the value of the input variable directly into the function to find the limit. This method works when the function is continuous at that point and doesn't result in an indeterminate form (e.g., 0/0 or ∞/∞).2.2.2 Factoring and CancellationFactoring and cancellation can be used to simplify expressions and eliminate common factors before applying direct substitution. This technique is particularly useful when dealing with polynomial functions.2.3 ContinuityA function is continuous at a point if the limit of the function exists at that point, the function is defined at that point, and the left and right limits are equal. A function is called continuous on an interval if it is continuous at every point within that interval.Chapter 3: Derivatives3.1 Introduction to Derivatives3.1.1 Definition of the DerivativeThe derivative of a function f(x) represents the rate at which the function changes with respect to its input variable. It is denoted as f'(x) or dy/dx and is defined as the limit of the difference quotient Δy/Δx as Δx approaches zero.3.1.2 Interpretation of the DerivativeThe derivative represents the slope of the tangent line to the graph of the function at a given point. It provides information about the rate of change, instantaneous velocity, and concavity of the function.3.2 Techniques for Finding Derivatives3.2.1 Power RuleThe power rule states that if f(x) = x^n, where n is a constant, then f'(x) = nx^(n-1). This rule allows us to find the derivative of polynomial functions.3.2.2 Chain RuleThe chain rule is used to find the derivative of composite functions. If y = f(g(x)), then dy/dx = f'(g(x)) * g'(x). This rule is particularly useful when dealing with functions that are composed of multiple functions.3.3 Applications of Derivatives3.3.1 Rate of ChangeThe derivative represents the rate of change of a function. It can be used to determine the instantaneous rate of change at a specific point or the average rate of change over a given interval.3.3.2 OptimizationDerivatives can be used to optimize functions by finding the maximum or minimum values. This involves finding the critical points where the derivative is zero or undefined and analyzing the behavior of the function around those points.Note: This is just a sample outline for the article on the answer key for an English advanced mathematics textbook. The actual content will depend on the specific exercises and problems in the textbook, which cannot be provided without access to the textbook itself.。
英语国家概况Chapter10

Foreign Language Teaching and Research Press
The Function of Congress
nations Biblioteka nd among states;Foreign Language Teaching and Research Press
The Function of Congress
– the power to declare war, raise and support armies, and provide and maintain a navy;
• 435 voting members plus a non-voting representative from Puerto Rico, the District of Columbia and four other territories of the U.S.; • State legislature divides each state into congressional districts, each district electing one Representative; • Qualifications for being a representative:
– over 30 years old;
– a US citizen for at least 9 years;
– resident in the state from which he is elected.
数学分析中的英文单词和短语

第一章实数集与函数数学分析中的英文单词和短语第二章数列极限Chapter 2 Limits of Sequences第三章函数极限Chapter 3 Limits of Functions第四章函数的连续性Chapter 4 Continuity of Functions第六章 微分中值定理及其应用Chapter 6 Mean Value Theorems of Differentials and their Applications第七章 实数的完备性Chapter 7 Completeness of Real Numbers第八章 不定积分Chapter 8 Indefinite Integrals第九章 定积分Chapter 9 Definite Integrals第十章定积分的应用Chapter 10 Applications of Definite Integrals第十一章反常积分Chapter 11 Improper Integrals 第十二章数项级数Chapter 12 Series of Number Terms第十三章函数列与函数项级数Chapter 13 Sequences of Functions andSeries of Functions第十四章幂级数Chapter 14 Power Series第十五章傅里叶级数Chapter 15 Fourier Series第十六章多元函数的极限与连续Chapter 16 Limits and Continuity of Functions of Several Variavles第十七章多元函数微分学Chapter 17 Differential Calculus of Functions of Several Variables第十八章隐函数定理及其应用Chapter 18 ImplicitFunciton Theorems and their Applications第十九章含参量积分Chapter 19 Integrals with Parameters第二十章重积分Chapter 20 Multiple Integrals第二十一章曲线积分Chapter 21 Curvilinear Integrals 第二十二章曲面积分Chapter 22 Surface Integrals。
Continuity and One-Sided Limits:连续性和片面的限制

Objectives:2.4.1 Describe continuity and be able to distinguish a continuous function from one with discontinuities.Materials:Exploration 2-4a (IRB)Lesson 1Warm Upp. 49-50: Quick Review: Q1-Q101. What is meant by the derivative of a function?2. What is meant by the definite integral of a function?3. Draw a pair of alternate interior angles.4. What type of function has a graph like the following:-4-3-2-112345-4-3-2-11234xy5. Sketch the graph of cos y x =6. Factor: 256x x +-7. Evaluate: 2001200053538. Evaluate: 5!9. No calculator! Divide 50 by ½ and add 3.Solutions:Q1. Instantaneous rate of changeQ2. Product of x and y , where x varies and y can vary 3.4. Exponential function5.-2π-3π/2-π-π/2π/2π3π/22π-5-4-3-2-112345xy6. x +6()x -1()7. 538. 1209. 103I. Continuity at a Point and on an Open IntervalA function is continuous if there are no holes, steps, or asymptotes. Basically, this means that you can draw the function without lifting your pencil. Some of the most interesting function s with discontinuities are “piecewise” functions.Graphing a Piecewise Function ManuallyPiecewise functions have multiple branches at different sections of the domain of the function. To manually graph a piecewise function, graph each piece separately and then erase the sections that are beyond the domain of that section.Example 1Manually graph the following piecewise function:24, 01, 0x x y x x -≤⎧=⎨+>⎩-4-3-2-112345-4-3-2-11234xyQuery: Is the function in Example 1 continuous? Solution: No, there is a step discontinuity.Example 2Manually graph the following piecewise function:f x ()=e x , x <11x -1,x ≥1⎧ ⎨ ⎩-4-3-2-112345-4-3-2-11234xyQuery: Is the function in Example 2 continuous? Solution: No, the vertical asymptote is a discontinuity.Graphing a Piecewise Function with a CalculatorGraph the function from Example 1, 24, 01, 0x x y x x -≤⎧=⎨+>⎩,by entering the following into Y 1: ()()()()24010x x x x -≤++>ExamplePlot the following function on your calculator: f x ()=x +4, x ≤2-x 2+8x -8, 2<x <5x +2, x ≥5⎧ ⎨⎪ ⎩ ⎪a. Does f(x) have a limit as x approaches 2?No, the function has different values if you approach from the left or from the right.b. Is f(x) continuous at x = 2? No, there is a gap.c. Does f(x) have a limit as x approaches 5?d. Is f(x) continuous at x = 5?“Types ” of Discontinuities: Holes, Steps, and AsymptotesThere are three basic types of discontinuities: holes, steps, and asymptotes.Figure 1: HoleFigure 2: Hole #2Not defined at x c =()lim x cf x → does not existFigure 3: StepFigure 4: Asymptote()()lim x cf x f c →≠Not defined at x c = and ()lim x cf x → does notexistDefinition of ContinuityA function is continuous at c if:()()lim x cf x f c →=.Continuous on an open interval (a, b): A function that is continuous at each point in the interval.Everywhere continuous: A function that is continuous on the entire real line (),-∞∞Removable Discontinuities:discontinuities that can be “removed” by redefining ()f c. Holes are removable discontinuities.Nonremovable Discontinuities: discontinuities that cannot be fixed easily.(1)Steps(2)Vertical asymptotesIdentifying DiscontinuitiesThe three types of discontinuities are easily identified by the cartoonish graphs found in the textbook. However, hole and jump discontinuities are invisible on graphing calculators. Therefore, you must be able to identify the discontinuities algebraically.1.Zeros in Denominators of Rational Functions: could be removable ornonremovable discontinuities.2.Holes in Piecewise Functions: these occur when there is a singular x-value that isnot in the domain of the function.3.Steps in Piecewise Functions: these occur when the endpoints of adjacentbranches don’t match up.4.Toolkit Functions: you must be familiar enough with the elementary functions tobe able to identify vertical asymptotes, i.e. tan x() and ln x().5.Plot with a Calculator: for unfamiliar functions, you may be able to identifyvertical asymptotes and steps by simply graphing the function. However,remember that holes cannot be seen on the graphs of calculators. Also, you may want to plot the functions in “dot mode” so that vertical asymptotes don’t appear to be part of the function.6.TABLE: If you suspect that there is a discontinuity at a particular x-value, checkthe table on your calculator. If an x-value has an ERROR, then there is adiscontinuity.Describing ContinuityOn tests and quizzes, you will be asked to “describe the continuity of a function”. The following are possible responses to this question:1.State that the function is “continuous everywhere”2.Identify the type of discontinuity and list the x-value(s) where the discontinuitiesexist.Put students into groups of either 4 or 5. Each student should plot two of the functions in the following set. Then, the students should take turn explaining the continuity of their functions. Students should show their calculators to their partners so that everyone understands the continuity description.Graph the following functions. State whether you think each of the functions is continuous for the entire real number line.a. 21y x =+ Continuousb. 12y x =- Discontinuity at x = 2.c. sin x y x = Appears to be continuous, but there is a discontinuity at 0x =.d. 242x y x -=+ Appears to be continuous, but there is a discontinuity at 0x =.e. y =1x, x ≠01, x =0⎧ ⎨ ⎩Discontinuity at x = 0.f. y =x 2-x -2x -2, x ≠21, x =2⎧ ⎨ ⎩Discontinuity at x = 2.g. y =tan x () Discontinuties at x =±π2,±3π2,... h. y =cos x ()Continuous everywhere i. y =x +5x -11Vertical asymptote at x = 11j. y =x +int sin πx ()Discontinuities at every integer (graph in dot mode) k. ()1p x x =There is a domain restriction and x = 0 (vertical asymptote). Nonremovable discontinuityl. ()21, 01, 0x x h x x x +≤⎧=⎨+>⎩Continuous on (),-∞∞m. ()211x v x x -=+Removable discontinuity at x = -1n. sin y x = Continuous on (),-∞∞o. ()232g x x x =-+ Continuous on (),-∞∞Which trigonometric functions are continuous on the entire number line? ()()sin and cos x x Query 2Define an interval for which the other trigonometric functions are continuous.tan():x 22,ππ- cot():x ()0,π sec():x ()22,ππ- csc():x 0,πSometimes you will be asked to discuss the continuity of a function for only a finite part of the number line (as opposed to the entire set of real numbers).Example 1Discuss the continuity of the following functions on the given interval:()3 [3,3]f t =--ContinuousHomework: Day 1: p. 50-51: 1-29 oddExit Ticket1. (T/F) If ()f c = L , then lim ()x cf x L →=.False. There could be a step discontinuity at x c =.Lesson 2Warm UpSketch 5 separate graphs that fit the following descriptions:1. has a value for f(-2) but has no limit as x approaches -22. is continuous at x = 4 and is “smooth” there3. has a value for f(2) and a limit as x approaches, but is not continuous at x = 2.4. the limit of f(x) as x approaches 5 is -2, and the value for f(5) is also -2.5. f(3) = 5, but f(x) has no limit as x approaches 3 and no vertical asymptote there.II. One-Sided Limits and Continuity on a Closed IntervalPreviously, we said that if a function approached different values from the left and right at a point x = c , then the limit did not exist there. Now, we will learn about limits that only consider one direction.Limit from the right: limit that only considers values greater than x = c .()lim x cf x -→Limit from the left: limit that only considers values less than x = c .()lim x cf x +→Figure 2: One-sided limitsExample 2Find the limit ()g x = as x approaches 5 from the left. 0The limit in Example 2 would be written like: 5lim 0x -→=Example 3: Step FunctionThe greatest integer function, or “step” function, has a series of gap discontinuities.Figure 3: Step functionFind the limit of the greatest integer function as x approaches 0 from the left and right.Limit from the left: -1 Limit from the right: 0In cases such as this, we say that the limit does not exist .***Limits Exist When:Definition of Continuity on a Closed IntervalA function f is continuous on the closed interval [],a b if it is:(1) continuous on the open interval (),a b and(2) ()()()()lim and lim x ax bf x f a f x fb +-→→==Condition (2) means that at each endpoint, the value of the function is equal to its limit from within the interval. In other words, there can be no jumps or gaps at the endpoints.Continuity on a Closed Interval can include functions such as ()f x =, which is not defined for x values less than zero. According to the definition, ()f x =on the interval [)0,∞Example 4Discuss the continuity of the function on the closed interval.()[] 5,5g x =-Example 5Discuss the continuity of the function on the closed interval.()[]123, 0, 1,43, 0x x f x x x -≤⎧=-⎨+>⎩Properties of ContinuityIf ()()and f x g x are continuous at x c =, then the following functions are also continuous at c .1. Scalar multiple: ()b f x ⋅2. Sum and difference: ()()f x g x ±3. Product: ()()f x g x ⋅4. Quotient:()()f xg xThere are certain functions that are always continuous at every point in their domain .Example 6Determine the domains of the example functions in Table 1. These functions are continuous everywhere in these domains.Table 1: Continuous FunctionsNote that these “continuous” functions are not necessarily continuous for all real numbers.Composite Functions are continuous if the individual functions are continuous. Example 7Discuss the continuity of the composite function ()()f g x .()() 1f x g x x==-()()f g x =This falls under Property of Continuity #4. The function is continuous over the domain: ()1,∞Example 8Discuss the continuity of the composite function ()()f g x .()()()2sin ; f x x g x x ==()()()2sin f g x x =This is clearly a continuous trigonometric function. The function is continuous over the domain: (),-∞∞Testing for ContinuitySometimes it is useful to define an interval over which a function is continuous. Consider the function ()()tan f x x =. It is not continuous for all real numbers, but we can define intervals for which it is continuous.The function has vertical asymptotes (non-removable discontinuities) at 322,,.x etc ππ=Interval for which ()()tan f x x = is continuous: ()22,ππ-Figure 4: ()()tan f x x =Homework: Day 2: p. 50-52: 11-19, 31-35 oddExit Ticket Questions1. Sketch the graph of any function f(x) such that()()33lim 1 and lim 0x x f x f x +-→→== Is the function continuous at 3x =? Explain.No, the function is not continuous at 3x =.。
高等数学双语教材答案

高等数学双语教材答案Chapter 1: Limits and ContinuitySection 1: Introduction to Limits and ContinuityThe concept of limits and continuity is fundamental in higher mathematics. In this section, we will introduce the basic definitions and properties associated with limits and continuity.1.1 Definitions of LimitsIn order to understand limits, we need to define what it means for a function to approach a particular value. Let f(x) be a function defined on an open interval containing c, except possibly at c. We say that the limit of f(x) as x approaches c is L, denoted bylim (x→c) f(x) = L, if for every ε > 0, there exists a δ > 0 such that |f(x) - L| < ε whenever 0 < |x - c| < δ.1.2 Basic Limit LawsOnce we have a clear understanding of limits, we can explore some basic laws that govern their behavior. These laws include the sum law, constant multiple law, product law, quotient law, and the power law.1.3 ContinuityA function f(x) is said to be continuous at a point c if three conditions are met: (1) f(c) is defined, (2) the limit of f(x) as x approaches c exists, and (3) the limit of f(x) as x approaches c is equal to f(c). We can also discuss continuity on an interval or at infinity.Chapter 2: DifferentiationSection 1: Introduction to DifferentiationDifferentiation is an important concept in calculus that allows us to find the rate at which a function is changing at any given point. In this section, we will introduce the concept of differentiation and its applications.2.1 Derivative DefinitionThe derivative of a function f(x) at a point c is defined as the limit of the difference quotient as h approaches 0. Mathematically, this can be written as f'(c) = lim (h→0) [(f(c + h) - f(c))/h].2.2 Differentiation RulesThere are several rules that allow us to find the derivative of a function quickly. These rules include the constant rule, power rule, sum rule, difference rule, product rule, quotient rule, and chain rule.2.3 Applications of DifferentiationDifferentiation has many applications in various fields, such as physics, economics, and engineering. It can be used to find maximum and minimum values, determine rates of change, and solve optimization problems.Chapter 3: IntegrationSection 1: Introduction to IntegrationIntegration is the reverse process of differentiation. It enables us to find the area under a curve and solve various mathematical problems. In this section, we will introduce the concept of integration and its applications.3.1 Indefinite IntegralsThe indefinite integral of a function f(x) is the collection of all antiderivatives of f(x). It is denoted by ∫ f(x) dx and represents a family of functions rather than a single value.3.2 Integration TechniquesThere are various techniques for finding antiderivatives and evaluating definite integrals. These techniques include basic integration rules, substitution, integration by parts, and trigonometric substitution.3.3 Applications of IntegrationIntegration has numerous applications, such as finding the area between two curves, calculating the length of curves, determining volumes of solids, and solving differential equations.ConclusionIn conclusion, the study of high-level mathematics, particularly limits, continuity, differentiation, and integration, is crucial for a comprehensive understanding of advanced mathematical concepts. This article has provided a brief overview of these topics, highlighting their definitions, properties, and applications. By mastering these concepts, students can develop strong problem-solving skills and apply them in various academic and real-world scenarios.。
国外经典教材高等数学答案

国外经典教材高等数学答案教材:Higher Mathematics - A Classic TextbookChapter 1: Limits and ContinuityExercise 1:1. Determine the limit of f(x) as x approaches 2, where f(x) = (x^2 + 3x - 10)/(x - 2).Solution:Using algebraic manipulation, we can simplify the function as follows:f(x) = (x + 5)(x - 2)/(x - 2)= x + 5As x approaches 2, we find that f(x) approaches 2 + 5 = 7.Exercise 2:2. Find the vertical and horizontal asymptotes, if any, for the functiong(x) = (3x^2 + 2)/(x + 1).Solution:To find the vertical asymptotes, we observe that g(x) approaches infinity as x approaches a value that makes the denominator, x + 1, equal to zero. Therefore, the vertical asymptote occurs at x = -1.To determine the horizontal asymptotes, we analyze the behavior of the function as x approaches positive and negative infinity. Using limits, we findthat both numerator and denominator are of the same degree (2). Thus, we divide the leading coefficient of the numerator by the leading coefficient of the denominator to determine the horizontal asymptote. In this case, the horizontal asymptote is y = 3.Chapter 2: DifferentiationExercise 1:1. Find the derivative of the function f(x) = 2x^3 - 3x^2 + 4x - 1.Solution:To find the derivative, we differentiate each term of the function separately:f'(x) = 2(3x^2) - 3(2x) + 4(1) - 0= 6x^2 - 6x + 4Exercise 2:2. Determine the critical points of the function g(x) = 3x^4 - 8x^3 + 6x^2 - 12x + 1.Solution:To find the critical points, we must find the values of x where the derivative of g(x) is equal to zero or undefined.Taking the derivative of g(x), we get:g'(x) = 12x^3 - 24x^2 + 12x - 12Next, we set g'(x) equal to zero and solve for x:12x^3 - 24x^2 + 12x - 12 = 0Using factorization or numerical methods, we find that x = 1 is a critical point.Chapter 3: IntegrationExercise 1:1. Evaluate the definite integral ∫(2x^2 + 5) dx from x = 1 to x = 3.Solution:To evaluate the definite integral, we first find the antiderivative of 2x^2 + 5:∫(2x^2 + 5) dx = (2/3)x^3 + 5x + CNext, we substitute the limits of integration:[(2/3)(3)^3 + 5(3)] - [(2/3)(1)^3 + 5(1)]= (2/3)(27) + 15 - (2/3) - 5= 18 + 15 - (2/3) - 5= 28 - (2/3)= 82/3Exercise 2:2. Compute the improper integral ∫(1/x) dx from x = 1 to x = ∞.Solution:To compute the improper integral, we evaluate the limit as the upper bound of integration approaches infinity:∫(1/x) dx = lim (n→∞) [∫(1/x) dx] from 1 to nIntegrating 1/x with respect to x, we obtain:∫(1/x) dx = ln|x| + CNext, we substitute the limits of integration and compute the limit as n approaches infinity:lim (n→∞) [ln|n| - ln|1|]= lim (n→∞) ln|n|= ∞Hence, the improper integral is divergent.This textbook provides comprehensive coverage of high-level mathematics, including limits, continuity, differentiation, and integration. By practicing these exercises and understanding the solutions, students can enhance their mathematical abilities and build a strong foundation in higher mathematics.。
1-2FindingLimits

x sin 1 2 lim x x 0 x With 2 2 2 ( ) 2 x sin 1 2 )2 1 2 1 lim( 1 . 2 x0 x 2 2 2
2
Ex.
sin x 1 x sin x x lim lim x 0 x 0 x sin x sin x 1 x
2 4 (2) 5 4 (2) 4
Indeterminate Forms
Indeterminate forms occur when substitution in the limit results in 0/0. In such cases either factor or rationalize the expressions. Ex.
x 3 x 3
b) lim f ( x) g ( x) lim f ( x) lim g ( x) x 3
4 (2) 6
2 f ( x) lim g ( x) 2 f ( x) g ( x) lim x 3 x 3 c) lim x 3 lim f ( x) lim g ( x) f ( x) g ( x) x 3 x 3
x 0
x 0.
Consider:
sin x y x
So guess:
What happens as x approaches zero? Graphically:
sin x lim 1 x 0 x
Looks like y→1
sin x Using the Sandwich theorem to find lim x0 x
0
1 lim
0
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
{(x, y)||xx0| , |yy0| , (x, y) (x0, y0)},
denoted as U °(A; ) or U °(A).
b. Relationship between point and point set:
2 2 (P , P ) ( x x ) ( y y ) . 1 2 1 2 1 2
y
P1(x1, y1)
P2(x2, y2)
x
O
Euclidean distance has the following properties:
(1) Positivity: (P,Q) ≥0; (P,Q)=0 P=Q; (2) Symmetry: (P,Q) = (Q, P); (3) Triangle inequality: (P,Q)≤ (P,S)+ (S,Q).
(iv) Accumulation point—If there exists point belong to any deleted neighborhood U °(A) of E, then A is called the accumulation point of E. Accumulation point itself may belong to E, or not belong to E.
Example 1. Assume D {(x, y)|1 x2 y2 4}, then the points, satisfy1 x2 y2 4, are the interior points of D; the points, satisfy x2 y2 4, are the boundary points of D, and they are all belong to D; the points, satisfy x2 y2 1, are also the boundary points of D, but they do not belong to D; DUD are the accumulation points of D. Open set—If every points of E belong to the interior of E(namely, int E E), then E is called an open set.
Basic Requirements
1.Master some concepts about the point sets in the plane, such as neighborhood, interiorpoint, boundary point, accumulation point, 0pen set, closed set, open domain, closed domain, bounded set, and unbounded set, etc. 2.To understand and describe the closed rectangle cover theorem on continuity of R2, finite cover theorem, accumulation principle, compactness theorem and Cauchy Convergence Criterion.
As any circular neighborhood of A can be contained in a square neighborhood of A, and vice versa, so it is often denoted circular neighborhood of A or square neighborhood of A indiscriminately as U(A; ) or U(A). Deleted neighborhood of A refers to:
When the Euclidean distance is introduced in R2, then it is called 2- dimensional Euclidean space, or 2dimensional metric space. The concept of neighborhood: We call the set of points {(x, y)|(xx0)2 (yy0)2 2} and {(x, y)| ||xx0 | , |yy0| } the -circular neighborhood with central A(x0, y0) and -square neighborhood with central A(x0, y0) , respectively.
The relationship between any point AR2 and any point set E R2: (i) Interior point—If there is a neighborhood U(A) of point A, such that U(A) E , then A is called the interior point of E; A set consists of all interior points of E is called interior of E, denoted as int E. (ii) Exterior point—If there is a neighborhood U(A) of point A, such that U(A)∩ E , then A is called the exterior point of E. (iii) Boundary point—If any neighborhood of the point A contains points in both E and Ec, then A is called the boundary point of E, namely, for any >0, formula U(A; )∩ E and U(A; )∩ Ec are always valid, where Ec R2 \ E. A set consists of all boundary points of E is called the boundary of E, denoted as E.
Examples of planar point sets 1.The first quadra: G = {(x, y)| x > 0, y>0}; 2. Lower half plane with X axis: H- = {(x, y)| y≤0};
3. Circle with central (x0, y0) and radius r:
§10.4 Taylor formula of two valued-function
§10.1 Multivariate Function
1. N-dimensional Euclidean space
2. The limit of points in R2 and continuity of R2 3. The concept of multivariate function
C ={(x, y)| (xx0)2 (yy0)2 = r 2}.
4. Disc with central (x0, y0) and radius r:
D = {(x, y)|(xx0)2 (yy0)2 < r 2};
5. Rectangle parallel to axis:
S={(x, y)|a≤ x ≤ b, c ≤ y ≤ d}=[a, b]×[c, d],
y b O
Point P(a,b)
number pair (a,b)
x a
Representation of points in plane: The points in plane is usually denoted by capital letters P, Q, X, Y etc. In order to emphasize its coordinates, they also can be expressed with P(x,y), Q(a,b),or Denoted as (x, y), (a, b) etc, directly.
C = {(x, y)| (xx0)2 (yy0)2 = r 2}.
Distance—The most basic concept
Euclidean Distance The Euclidean Distance between P1(x1, y1) and P2(x2, y2) in coordinate plane is defined by
(v) Isolated point—If AE, but not the accumulation point of E, namely, there exists a >0, such that U °(A; )∩E =, then A is called the isolated point of E.
3.Master the concept of two valued function and its limit.
a. Basic concept Coordinate Plane: The plane which is decised a coordinate system is called Coordinate Plane, denoted by R2. It makes 1-1 correspondence between the real number pair and the plane point.
Obviously, the isolated point must be a boundary point; The interior point and non-isolated boundary point must be the accumulation point; If the point is neither accumulation point nor isolated point, then it must be to the exterior point.
