Two-Photon Emission from Semiconductors

合集下载

胆甾相液晶激光器研究进展

胆甾相液晶激光器研究进展张世超;黄玉华;王辉【摘要】Cholesteric liquid crystal lasers have been extensively studied but barely studied in our country.The periodic helical structure and selective reflection property of cholesteric liquid crystal were introduced.The principle and research progress of cholestenc liquid crystal based lasers and its potential applications were reviewed to provide some reference for the specialists in our country.%近年来国外学者对胆甾相液晶激光进行了大量研究,而国内在这方面研究甚少.介绍了胆甾相液晶周期性螺旋结构及其选择性反射特性,以及胆甾相液晶激光的工作原理,总结了胆甾相液晶激光的发展现状及其潜在的应用前景,以期为国内液晶研究的同行提供一点参考.【期刊名称】《激光技术》【年(卷),期】2011(035)004【总页数】4页(P514-517)【关键词】激光器;胆甾相液晶;液晶激光器;光子带隙【作者】张世超;黄玉华;王辉【作者单位】浙江师范大学,信息光学研究所,金华,321004;浙江师范大学,信息光学研究所,金华,321004;浙江师范大学,信息光学研究所,金华,321004【正文语种】中文【中图分类】O43;TN248引言光子晶体是一种介电常数呈周期性变化的介电质，会形成光子禁带和导带，光子在导带里可以顺利传播，在光子禁带内则会被禁止。

这一特点使得光子晶体可以应用于很多领域，尤其在集成光学具有巨大的应用前景，因此引起了广泛的研究兴趣。

自动往返电动小汽车(毕业设计)

一. 毕业实践任务书无锡职业技术学院毕业实践任务书课题名称：自动往返电动小汽车指导教师：XXXXXXX 职称：讲师指导教师：职称：专业名称：XXXXXXXX 班组：XXXXXX学生姓名：XXXXXXX 学号：05一. 课题需要完成的任务：设计并制作一个能自动往返于起跑线与终点线间的小汽车。

允许用玩具汽车改装，但不能用人工遥控（包括有线和无线遥控）。

图1跑道顶视图跑道宽度0.5m，表面贴有白纸，两侧有挡板，挡板与地面垂直，其高度不低于20cm。

在跑道的B、C、D、E、F、G各点处画有2cm宽的黑线，各段的长度如图1所示。

设计要求1、车辆从起跑线出发（出发前，车体不得超出起跑线），到达终点线后停留10秒，然后自动返回起跑线（允许倒车返回）。

往返一次的时间应力求最短（从合上汽车电源开关开始计时）。

2. 达终点线和返回起跑线时，停车位置离起跑线和终点线偏差应最小（以车辆中心点与终点线或起跑线中心线之间距离作为偏差的测量值）。

D～E间为限速区，车辆往返均要求以低速通过，通过时间不得少于8秒，但不允许在限速区内停车。

二. 课题计划：2006.3.3~2006.3.6 熟悉课题，可行性方案分析及方案论述。

2006.3.7~2006.3.19 查阅资料，设计各部分硬件。

2006.3.19~2006.4.10 画原理图，印刷线路板。

2006.4.10~2006.4.20 编写程序验证部分硬件。

2006.4.21~2006.4.25 写出毕业论文。

计划答辩时间：4.21-4.28XXXXX 系（部、分院）2006年02年18日二.外文翻译VIDEOCASSETTEBefore the videocassette recorder there was the movie projector and screen. Perhaps you remember your fifth-grade teacher pulling down a screen—or Dad hanging a sheet on the wall, ready to show visiting friends the enthralling account of your summer vacation at the shore. Just as the film got started, the projector bulb often blew out.Those days did have one advantage, though: the screen was light, paper-thin and could be rolled into a portable tube. Compare that with bulky television and computer screens, and the projector screen invokes more than just nostalgia. Could yesterday's convenience be married to today's technology?The answer is yes, thanks to organic light-emitting materials that promise to make electronic viewing more convenient and ubiquitous. Used in displays, the organic materials are brighter, consume less energy and are easier to manufacture (thus potentially cheaper) than current options based on liquid crystals. Because organic light-emitting diodes (OLEDs) emit light, they consume significantly less power, especially in small sizes, than common liquid-crystal displays (LCDs), which require backlighting. OLEDs also offer several exciting advantages over common LEDs: the materials do not need to be crystalline (that is, composed of a precisely repeating pattern of planes of atoms), so they are easier to make; they are applied in thin layers for a slimmer profile; and different materials (for different colors) can be patterned on a given substrate to make high-resolution images. The substrates may be inexpensive glass or flexible plastic or even metal foil.In the coming years, large-screen televisions and computer monitors could roll up for storage. A soldier might unfurl a sheet of plastic showing a real-time situation map. Smaller displays could be wrapped around a person's forearm or incorporated into clothing. Used in lighting fixtures, the panels could curl around an architectural column or lie almost wallpaperlike against a wall or ceiling.LEDs currently have longer lifetimes than organic emitters, and itwill be tough to beat the widespread LED for use in indicator lamps. But OLEDs are already demonstrating their potential for displays. Their screens put out more than 100 candelas per square meter (about the luminance of a notebook screen) and last tens of thousands of hours (several years of regular use) before they dim to half their original radiance.Close to 100 companies are developing applications for the technology, focusing on small, low-power displays [see box on page 80]. Initial products include a nonflexible 2.2-inch (diagonal) display for digital cameras and cellular phones made jointly by Kodak and Sanyo, introduced in 2002, and a 15-inch prototype computer monitor produced by the same collaborative venture. The global market for organic display devices was about $219 million in 2003 and is projected to jump to $3.1 billion by 2009, according to Kimberly Allen of iSuppli/Stanford Resources, a market-research firm specializing in displays.一、What LED to OLEDCRYSTALLINE semiconductors—the forerunners of OLEDs—trace their roots back to the development of the transistor in 1947, and visible-light LEDs were invented in 1962 by Nick Holonyak, Jr. They were first used commercially as tiny sources of red light in calculators and watches and soon after also appeared as durable indicator lights of red, green or yellow. (When suitably constructed, LEDs form lasers, which have spawned the optical-fiber revolution, as well as optical data storage on compact discs and digital video discs.) Since the advent of the blue LED in the 1990s [see “Blue Chip,” by Glenn Zorpette; Scientific American, August 2000], full-color, large-screen television displays made from hundreds of thousands of LED chips have appeared in spectacular fashion on skyscrapers and in arenas [see “In Pursuit of the Ultimate Lamp,” by M. George Crawford, Nick Holonyak, Jr., and Frederick A. Kish, Jr.; Scientific American, February 2001]. Yet the smaller sizes used in devices such as PDAs (personal digital assistants) and laptops are not as practical.LEDs and OLEDs are made from layers of semiconductors—materials whose electrical performance is midway between an excellent conductorsuch as copper and an insulator such as rubber. Semiconducting materials, such as silicon, have a small energy gap between electrons that are bound and those that are free to move around and conduct electricity. Given sufficient energy in the form of an applied voltage, electrons can “jump” the gap a nd begin moving, constituting an electrical charge. A semiconductor can be made conductive by doping it; if the atoms added to a layer have a smaller number of electrons than the atoms they replace, electrons have effectively been removed, leaving positively charged “holes” and making the material “p-type.” Alternatively, a layer that is doped so that it has an excess of negatively charged electrons becomes “n-type” [see box on opposite page]. When an electron is added to a p-type material, it may encounter a hole and drop into the lower band, giving up an amount of energy (equal to the energy gap) as a photon of light. The wavelength depends on the energy gap of the emitting material.For the production of visible light, organic materials should have an energy gap between their lower and higher conduction bands in a relatively small range, about two to three electron volts. (One electron volt is defined as the kinetic energy gained by an electron when it is accelerated by a potential difference of one volt. A photon with one electron volt of energy corresponds to the infrared wavelength of 1,240 nanometers, and a photon of two electron volts has a wavelength half as much—620 nanometers—a reddish color.)二、A Surprising GlowORGANIC semiconductors are formed as aggregates of molecules that are, in the technologies being pursued, amorphous—a solid material, but one that is noncrystalline and without a definite order. There are two general types of organic light emitters, distinguished by “small” and “large” molecule sizes. The first practical p-n-type organic LED, based on small molecules, was invented in 1987 by Ching W. Tang and Steven A. Van Slyke of Eastman Kodak, after Tang noticed a surprising green glow coming from an organic solar cell he was working on. The duo recognized that by using two organic materials, one a good conductor of holes and the other a good conductor of electrons, they could ensure that photon emission would take place near the contact area, or junction, of the two materials, as in acrystalline LED. They also needed a material that held its electrons tightly, meaning that it would be easy to inject holes. For the light to escape, one of the contacts must be transparent, and the scientists benefited from the fortunate fact that the most widely used transparent conducting material, indium tin oxide, bound its electrons suitably for p-type contact material.The structure they came up with has not changed much over the years and is often called “Kodak-type,” because Kodak had the basic patent [see box on opposite page]. Beginning with a glass substrate, different materials are deposited layer by layer. This process is accomplished by evaporating the constituent materials and letting them condense on the substrate. The total thickness of the organic layers is only 100 to 150 nanometers, much thinner than that of a conventional LED (which is at least microns in thickness) and less than 1 percent of the thickness of a human hair. Because the molecules of the materials used are relatively lightweight—even lighter than a small protein—the Kodak-type OLEDs are referred to as “small molecule” OLEDs.After their initial insight, Tang and Van Slyke tinkered with the design to increase efficiency. They added a small amount of the fluorescent dye coumarin to the emitter material tris (8-hydroxy-quinoline) aluminum. The energy released by the recombination of holes and electrons was transferred to the dye, which emitted light with greatly increased efficiency. Deposition of additional thin layers of indium tin oxide and other compounds next to the electrodes altered the interaction of the thicker layers and also improved the efficiency of the injection of holes and electrons, thereby further upping the overall power efficiency of the fluorescent OLED.Organic LEDs of this small-molecule type are used to make red, green and blue light, with green light having the highest efficiency. Such green-emitting OLEDs can exhibit luminous efficiencies of 10 to 15 candelas per ampere—about as efficient as commercial LEDs today—and seven to 10 lumens per watt, values that are comparable to those for common incandescent lamps.录像机在卡匣式录像机出来之前，我们用的是电影放映机与屏幕。

7. Emission and Absorption

abs dPki = Bki wν (ν) (7.2) dt is proportional to the spectral energy density wν (ν) = n (ν)h ν of the radiation ﬁeld (where n (ν) is the number of photons h ν per unit volume within the frequency interval ∆ν = 1 s−1 ). The proportionality factor Bki is the Einstein coefﬁcient for absorption. Each absorption takes one photon from a speciﬁc mode of the radiation ﬁeld (see Sect. 3.1) and therefore decreases the number of photons in this mode by one. The radiation ﬁeld can also induce atoms in an excited state with energy E i to emit a photon with energy h ν = E i − E k into a speciﬁc mode of the radiation ﬁeld and to go into the lower state E k . This process is called induced (or stimulated) emission. It increases by one the number of photons in this mode from which the inducing photon came. Since the two photons are in the same mode, they have identical propagation directions. The energy of the atom is reduced by ∆ E and that of

光电英语词汇汇总

光电英语词汇汇总photographic reconnaissance 照相侦察photographic recorder 摄影记录器，录相器photographic refractor 天体折射照相仪photographic reproduction 照相复制法photographic sensitometer 感光度测定仪photographic snesitivity 感光度photographic sound recorder 光录声机photographic standard irradiance 照相标准辐照度photographic tansmission density 照相透光密度photographic zenith tube 照光天顶管photography 照相术，摄影术photogravure 照相凹板印刷photogroduct 光化产品photogun 光电子枪photohalide 感光卤化物photohead 光电传感头photoheliograph 太阳照相机photoheliography 太阳照相术photohole 光穴photohyalography 光照蚀刻术photoimpact (1)光冲量(2)光控脉冲photoinactivation 光纯化作用photoinduced 光致的，光感生的photoinduced strain 光致应变photoinduction 光诱导，光感生photointaglio 照相板，影印版photointerpreter 相片判读装置photoionization 光致电离photoionization detector 光电离检测器photoionization threshold 光致电离阈photoisomer 光致同分异体photoisomerisom 光致同分异构photojunction cell 光电二极管photojunction diode 光电二极管photoklystron 光电速调管photolabile 对光不稳的photolayer 光敏层photolesn chromatism 摄影透镜色差photoline 光线photolithgraphic 光刻法的photolithography 光蚀刻微影; 光刻法; 平印照相制板photolocking 光锁定photology 光学photoluminescence 光致发光photoluminescence efficiency curve 光致发光效率曲线photolysis 光解作用photolyte 光解质photolytic 光解的photom 光度学photomacrogaph 宏观照片photomagnetic 光磁的photomagnetic effect 光磁效应photomagnetoelectric 光磁电的photomagnetoelectric effect 光磁电效应photomap 空中摄影地图photomask 光掩模photomask materials 光罩材料photomation 自体摄印相机photomatrices 光矩阵photomechanical 光学机械的photometallic etching 光金属蚀刻photometallurgical microscope 金相摄影显微镜photometeor 发光陨星photometer 光度计photometer bench 光度计座photometer head 光度计头photometers 光度计photometric 光度的photometric brightness 光亮度photometric brightness scale 光亮度标photometric calibration 光度校正photometric calibration data files 光度校准数据文件photometric computer 测光计算机photometric head 光度头photometric integrating sphere 光度积分球photometric method 光度测定法photometric paradox 光度佯谬photometric parallax 光度视差photometric period 光度周期photometric quality 光度质量photometric quantity 光度值，光度量photometric receiver 光度计photometric relationship 光度关系photometric repartition 光度分布photometric scale 光度标photometric standard 光度标准photometric standard lamp 光度标准灯photometric sterance 光辐通量photometric term 光度学术photometric test 光度检查photometric test plate 光度试验板photometric transfer function 光度传逊函数photometric units 光度学单位photometric wedge 测光楔photometry 光度学，光度测量术photomicrograph (1)显微照置(2)显微照片photomicrographic apparatus 显微照相装置photomicrographic tungsten arc lamp 显微照相钨弧光灯photomicrography 显微照相术photomicrometer 显微光度计photomicroscope 照相显微镜photomixer 光混频器photomixer diode 光混频二极管photomixing 光混频photomixing device 光源频器photomixing technique 光混频技术photomodulator 光调制器photomontage (1)光片剪辑(2)合成照片photomosaic 感光镶嵌幕photomotion 光激活动photomultiplier 光电倍增管photomultipliers 光电子增倍管(PMT)photomuon 光μ介子photon 光子photon absorption 光子吸收photon activated switch 光子起动开关photon amplification 光子放大photon annihilation 光子湮没photon avalanche 光子雪崩photon beam 光子束photon bombardment 光子轰系photon bunching 光子聚束photon capture 光子俘获photon counter 光子计数器photon counting spectrophotometer 光子计数分度计photon density 光子密度photon detector 光子探测器photon drag detector 光子牵引探测器photon drag photodetector 光子牵引光探测器photon effect 光子效应photon emission 光子发射photon excited atom 光子激发原子photon exitance 光子出photon fluctuation 光子起伏，光子波动photon flux 光子通量photon intensity 光子强度photon irradiance 光子辐射度photon liberation 光子逸出photon lifetime 光子寿命photon limited sensitivity 光子限灵敏度photon nosie 光子噪声photon radiance 光子辐射强度，光子辐射率photon statistics 光子统计学，光子流统计分布photon steradiance 光子辐射强度photon stream 光子流photon structure 光子结构photon-generated carrier 光生载流子photon-induced 光子感生的photon-induxed action 光子感生作用photonegative effect 负光电效应photonephelometer 光电浊度计photoneutron 光激中子photoneutron emission 光激中子发射photonic band structures 光子能带结构photonic band-gap (PBG) system 光能隙系统photonic bandgap 光子能隙photonic crystal 光子晶体photonic crystal fiber 光子晶体光纤photonic crystals 光子晶体Photonics 光电photonoise limit 光子噪声限photontransition 光子跃迁photonuclear disintegration 光致核蜕变photonuclear excitation 光核激发photooxidation 感光氧化用，光致氧化作用photooxygenation 光致氧化photooxygenation actinometer 光致氧化感光计photopeak 光巅，光峰photopen recorder 光笔记录器photoperiod 光周期photoperspectograph 摄影透视仪photophase 光相位photophobia 畏光症photophone 光电话，传真电话photophoresis 光致漂移photophysics 光子物理学photopia 光适应photopic eye 适光眼photopic range 适光范围photopic vison 亮视觉，白画视觉photoplane 摄影飞机photoplastic effect 光塑性效雁photoplastic material 光塑材料photoplastic recording 感光塑料记录photoplate 照相底片photopolymer 光聚合物photopolymer holography 光聚合物全息术photopolymerization 光聚作用photopositive 照相正片photopredissociation 光致预离解photoprigment 感光色素photoprint 影印，照页复制photoprocess 光学处理，光学加工photoproduction 光致作用，光生photoproducton cross-section 光致作用截面photoptometer 光敏计，光觉计photoptometry 辨光测验术photoradar 光雷达photoradiogram 光电传真电报photoransmitter 照片传真发器photoreaction 光致反应photoreader 光电读出器photoreading 光读婺photoreceiver 照片传真接器，光接收器photoreceptor 光感受器photorecombination laser 光复合激光器photoreconnaissance 摄影侦察photoreconversion 光致再转换photorecorder 摄影记录器，自动照明记录器photorectifier 光电检波器photoreduce 照相缩小photoreduction (1)光致还原(2)照相缩版photorefraction 光折射photorefractive 光致折变的photorefractive effect 光折射效应photorelay 光继电器photorepeater 复印机photoresist optics exposure 光电导曝光photoresistance 光敏电photoresistor 光敏电阻photoresistor-cell relay 光敏元件继电器photoresponse 光响应photoresponsive retina 光响应视网膜photoresponsive tube 光响应管photorproton 光质子photoscanning 光扫描photoscope 透视镜photosensing units 光感测元件photosensitiser 感光剂，光敏剂photosensitive 光感的，感光的photosensitive area 光敏区，光敏面积photosensitive device 光敏器件photosensitive film 光敏膜photosensitive glass 光敏玻璃photosensitive layer 光敏层photosensitive material 感光材料photosensitive medium 光敏媒质photosensitiveness 光敏性，光敏度photosensitivity 光敏性，感光性，感光灵敏度photosensitization 光敏作用photosensitizer 光敏剂photosensor 光敏器件photoseparation 光分离photoset 照相版排photosignal 光信号photosource 光源photosphere 光球层photospot 聚光photostability 耐光性photostable 不感光的，耐光的photostar 发光星体photosterograph 立体测图仪photostimulaton 光剌激photostudio (1)照相馆(2)摄影棚photosurface 光敏面photosurface spectral response 光敏面光谱响应photoswitch 光开关photosynthesis 光合作用phototaxis 趋光性phototelegram 传真电报phototelegraph 传真电报phototelegraphy 传真电报术phototelephone 传真电报机phototelephony 传真电话术phototelescope 照相望远镜phototherapeutics 光线治疗学phototherapy 光线疗法photothermionic image converter 光热离子变相管phototimer (1)曝光计(2)摄影计时器(3)延时光控继电器phototiming 光同调步phototransistor 光电晶体管，光敏三极管phototransistors 光电晶体phototransmutation 感光蜕变phototriode 光电三极phototroller 光控电管phototron 矩阵光电管phototrop 光色互变phototropic 向光的phototropic vision 摄影仪phototropism (1)趋光性，向光性(2)光色互变现象phototube 光电管phototubes 光电管phototype 珂罗版phototypesetter 照相排字机photounit 光电件photovalue 光阀photovaoltaic 光生伏打的photovisual objective 拟视照相物镜photovisula achromatism 光化视觉消色差性photovolt desitometer 光电密度计photovoltage 光电压photovoltaic 光生伏打photovoltaic cell 光生伏打电池photovoltaic converter 光伏变换器，光电能量变换器photovoltaic effect 光生伏打效应photovoltaic infrared detector 光伏红外探测器photox cell 氧化亚铜光电元件photoxide 光氧化物photozincograph 照相锌版photsyntometer 光合计phrolysis 高温分解，热解phromagnetic 热磁的phromagnetic detection 热磁探测phse-modulated signals 调相信号phse-modulated waves 调相波phthalocyanin 屻花悄phtoochromatic filter 光致变色滤光器physi-optics 物理光学physical absorption 物理吸附Physical chemistry 物理化学physical constraint 物理约束physical development 物理显影physical entropy 物理熵physical interpretation 物理解释physical optics 物理光学physical photometer 物理光度计physical photometry 物理光度测量学physical quantity 物理量physical thickness 物理厚度physics 物理学physiological 生理的physiological blur 生理模糊斑physiological model 生理模型physiological optics 生理光学physiological photometer 生理光度计physiology 生理学physioptial 物理光学的pic (1)图片，照片(2)图画，图像pic-up device 摄像装置pick-off diode 截止二极管pick-off gear 可互换齿轮pick-up (1)拾波，拾声(2)拾波器(3)拾声器(4)传感器(5)电视摄像管pick-up camera 摄像机pick-up head lenses 光碟机读取头透镜pick-up system 摄像系械pick-up tube 摄像管pickoff (1)传感器，探测器(2)截止(3)拾取pickoff couple 截止耦合器pickoff unit (1)接收器(2)截止器piclear unit 图像清除器picling (1)酸浸(2)浸渍picnometer (1)比重瓶(2)比色计pico 皮picosecond (ps)微微秒picosecond light pulse 微微秒光脉冲picosecond pulse 微微秒脉pictorial display 图像显示pictorial holography 图像全息术pictorial infrared photography 红外图像照相术pictorial view 示图，插图picture (1)画，图像(2)图片，照片picture bit rate 图像位速率picture element 像素，像元picture field 图像场picture frequency 图像频率picture image 图像picture jump 图像跳动picture pattern 图像，图样picture processing 图像处理picture quantizer 图像数字转换器picture retention 图像残留picture signal 图像信号picture signal amplitude 图像信号振幅picture synchronization 图像同步picture taking wavelength 摄影波长picture transmission camera system 图像传输摄影机系统picturephone 电视电甘picturephone set 电视电话机piecewise interferometric generation 分段傅里叶谱pieometer 压力计，压强计piercing 分段干涉量度振荡piezo 钻孔piezo-luminescence 压难发光piezo-optical coefficient 压光系数piezo-optical property 压光性质piezocrystal 压，压力piezoelectric 压电晶体piezoelectric ceramic 压电的piezoelectric constant 压电陶瓷piezoelectric crystal 压电常数piezoelectric effect 压电晶体piezoelectric laser modulation 压电现像，压电学piezoelectric light modulator 压电激光调制piezoelectric matrix 压电光调制器piezoelectric positioning equipment 压电定位设备piezoelectric quartz crystal 压电矩阵piezoelectric resonator 压电石英晶体piezoelectric tensor 压电共振器piezoelectric transducer 压电换能器piezoelectric transformer 压电变压器piezoelectric vibration 压电振动piezoelectricity 压电效应piezoid 压电石英piezometric 量压的，测压的piezometry 压力测定piezoquartz 压电石英piezoresistance 压敏电阻piezoresistive 压缩电阻的piezoresistor 压敏电阻器pig iron 生铁pigment (1)色素(2)颜料pigment epithelium 色素层pile-of-plates polarizer 玻片堆偏振器pile-up (1)堆积效应(2)堆集，聚集，积累pillar 柱，墩pillbox antenna 抛物柱面天线pillbox distribution 抛物柱面分布pillow (1)枕块(2)轴枕pilot ballon theodolite 气球测风经纬仪pilot lamp 指示灯pilot light 指示灯pilot wave 领波pimpling 凸起pin (1)别针(2)销钉(3)插头(4)管脚pin clamp 插销口pin photodiode 针状光电二极管pin photodiode modules for communication 通信用PIN光二极体模组pin photodiodes for communication 通信用PIN光二极体pin-camera 针孔照相机pin-electrode 针状电极pin-electrode discharge technique 针极放电技术pinacoid 轴面pinch 箍缩pinch effect 箍缩效应pinch laser 箍缩激光器pinch plasma 箍缩等离子体pinch pump 箍缩泵pinch-discharged-pumped laser 箍缩收电抽运激光器pinch-off effect 箍断效应pinch-off voltage 箍断电压pinch-preheated 收缩预热的pincushion aberration 枕形像差pincushion distortion 枕形失真pincushion-shaped image 枕形像pinhole 针孔，小孔pinhole camera 针孔照相机pinhole filter 针孔滤波器pinhole filters 针孔滤光镜pinhole image 针孔像pinhole imaging 针孔成像pinhole photography 针孔照相术pinhole system 针孔系统pinholes 针孔pinion 小齿轮，副齿轮pinion shaft 齿轮轴pink 淡红色的，纷红色的pinning (1)阻塞(2)闭合pinor 自旋量pinpoint (1)针尖(2)空中精确摄影(3)航空照片(4)精确定位pinpoint accuracy 高精确度pinpoint technique 精密技术pinpointed focus 定位焦点pint 品脱pip (1)针头(2)反射点(3)针头信号(4)广播报时信号pipe (1)管，导管(2)管状物pipe cross 十字接头，交接头pipeline (1)管道(2)输送管piping (1)管系(2)导管piquant 图佣数字转换器Pirani gage 皮剌尼计piston 活塞pit 凹痕，麻点pitch (1)螺距，齿节间距(2)沥青(3)松鲁(4)音调pitch adhesive 沥青黏结剂pitch angle 俯仰角pitch control (1)色调控制(2)音调控制pitch error (1)螺距误差(2)周节误差pitch gage (1)螺距规(2)周节仪pitch lap 沥青抛光盘pitch line (1)中心线(2)分度线pitch polishing tol 沥青抛光模pitch viscosity 沥青黏度pitting (1)凹痕，麻点(2)锈斑pivot (1)枢纽(2)支轴(3)支点pivot bearing 枢轴承pivot lesn apparatus 旋转透镜装罝pivot pad 枢轴垫，心轴垫pivot screw 枢轴螺钉pivot-point 支点pivoted axle 转动轴，摆动轴pixel 像素，像元placement 方位，部位，位置Placideo's disk 浦拉西多盘，角膜镜plain bearing 普通轴承，滑体轴承plain view drawing 平面图plan (1)计划(2)平面图，设计图plan of site 总布置图plan view 平面图，俯视图planar access couple 平面存取耦合器planar array 平面阵列planar dielectric waveguid 平面介质波导planar epitaxial phototransistor 平面外延光电晶体管planar hologram 平面全息图planar iris 平面可变光阑Planar lens 普拉纳镜头planar mirror 平面反射镜planar mosaic array 平面镶嵌阵列planar optical waveguide 平面光波导planar phottransistor 平面光电晶管planar rotor 平面转动片planar stripe DH laser 平面条状DH激光器planar waveguide-type 平面波导型planar-diffused transistor 平面扩散晶体管Planck function 普朗克函Planck's blackbody law 普朗克黑体定定律Planck's constant 普朗克常数Planck's holhrum radiaton 普朗克空腔辐射Planck's law 普朗克定徭Planck's oscillator 普朗克振子Planck's radiation law 普朗克辐射定且Planck's spectral radiation formula 普朗克光谱辐射公式Planckian locus 普朗克轨迹Planckian radiator 普朗克辐射体plane (1)平面(2)程度，水平(3)飞机(4)平面的plane angle 平面角plane cam 平面凸轮plane cocular 平场目镜plane goniometer 平面测向计plane grating 平面光栅plane hologram 平面全息图plane interference 平面干涉plane mirror 平面反小镜plane monchromator 平面色器plane of field lens 场镜面plane of incidence 入射面plane of oscillation 振动面plane of polarization 偏振面plane of projection 投影面plane of reflection 反射面plane of refraction 折射面plane of sharpest focus 最锐聚焦面plane of symmetry 对面plane of vibration 振动面plane optical flat 平面光学平晶plane polariscope 平面偏振镜plane polarization wave 面振波plane polarized (1)面振的(2)平面极化的plane polarized laser beam 平面偏振激光束plane polarized wave 面振波plane polarizedl ight 面振光plane position indicator (PPI)平面位置指示器，平面示伅器plane resonator 平面型共振器plane rod end 棒端面plane section 剖面plane shape irdome 平面型红外整流罩plane shutter 平面快门plane spatial filter 平面空间滤波器plane surface 平面plane surveying 平面测量plane wave 平面波plane wave front 平面波前plane-grating spectrograph 平面光栅摄谱仪plane-paralledl cavity 平行平面共振腔plane-parallel mirror 平行平面反射镜plane-parallel resonator 平行平面共振器plane-table alidade 平板照准仪plane-table survey 平板测planeness 平度planet 行星planetarium (1)天文馆(2)天象仪(3)太阳系仪planetarium projector 天象投影仪planetary (1)行星的(2)行星齿轮的planetary mission 行星齿轮变速管planetary pinion 行星小齿轮planetary radiation 行星辐射planetray microfilmer 文献资料翻拍机planetray reducer 行星减速齿轮planigraphy 层析X射线照相法planimegraph 面积比例规，缩图器planimeter (1)面积仪(2)求积仪planimetry 测面学，平面几何planitron 平面数字管planizer 平面授描头，平面发生器plank 板，厚板plano-aspheric corrector 平板非球面校正镜「施密特校正镜plano-aspheric transmitting corrector 平面非球面透射校正镜plano-concave lens 平凹透镜plano-convcave 平凹的plano-convex 平凸plano-convex lens 平凸透镜planometer 测平面仪，平面规planopaallel plate 平行平面板，平行平晶planoscope eyepiece 平场目刽planox 平面氧化的plant (1)成套设备(2)站(3)工厂，车间plariser 偏振器，偏振片plasma 等离子体，等离子区plasma -speetrometer 等离子光谱仪plasma arc pumping 等离子体电弧抽运plasma channel 等离子体通道plasma column 等离子体柱plasma corona 离子体电晕plasma CVD equipment 电浆CVD设备plasma density profile 等离子密度分布plasma dignostics 等离子体诊断Plasma Display Panel (PDP)电浆显示器plasma display panels (PDP)电浆显示器plasma ejection 等离子体抛射Plasma Enhance Chemical Vapor Deposition (PECVD)电浆增强式化学气相沉积法plasma exciatiaon 等离子体激发plasma focus 等离子焦点plasma frequency 等离子体频率plasma generation with laser 激光产生等离子体plasma generator 等离子体发生器plasma laser 等离子体激光器plasma light source 等离子体光源plasma oscillation 等离子体振荡plasma parameter 等离子体参数plasma pinch pump 等离子体箍缩泵plasma polymerization 等离子体聚合plasma polymerized coating 等离子体聚合涂层plasma polymerized thin film 等离子体合薄膜plasma radiation 等离子体辐射plasma reflectivity 等离子体反射率plasma slab 等离子层plasma spatial filter 等离子体空间滤光片plasma sphere 等离子层plasma torch 等离子火焰plasma tube 等离子体管plasma wave 等离子体波plasma-overlap laser 等离子体并行激光器plasma-pump laser 等离子体泵激光器plasma-sheet CO2 laser 等离子体薄板CO2激光器plasmat-type copying lens 等离子型仿徵透镜plasmatron 等离子管，等离子流发生器plasmoid 等离子体粒团plasmon 等离子体激元plaster (1)熟石膏(2)硬膏plaster block 石膏镜盘plaster of Paris 熟石膏，烧石膏plastic (1)塑料的(2)塑性的，范性的(3)塑料plastic aspheric lenes 塑胶非球面透镜plastic cement 塑料胶plastic clad fiber 塑料色层纤维plastic deformation 塑或变形plastic dye laser 塑料染料激光器plastic ferrules 塑胶箍(套管)plastic fibre optics 塑料纤维光学plastic film 塑料薄膜plastic filters 塑胶滤光镜plastic focusing fibre 塑料聚焦纤维plastic laser 塑料激光器plastic lens system 塑料透镜系统plastic material (1)塑料(2)可塑材料，塑性材料plastic optical fiber 塑料光学纤维plastic optics 塑料光学纤维plastic polishing 成型抛光plastic Q switch 塑料Q开关plastic recoding medium 塑性记录媒质plastic shim material 塑性垫片材料plastic spectacle lens 塑料眼镜片plastic spheric lenes 塑胶球面透镜plastic-base mirror 塑料底座镜plasticien 代用黏土plasticity (1)可塑性，范性(2)黏性plasticization 增塑plasticizer (1)增塑剂(2)增韧剂plastics 塑料，塑胶plasto-elasticity 弹塑性力学plastyle lens 塑玻透镜plate (1)盘(2)片(3)薄反(4)板极(5)底片plate camera 干板照相机plate glass 玻璃板plate holder 干板照拿plate let laser 小片激光器plate level 盘式水准器plate level vial 盘水准管plate library 底片库plate object 板状plate potential 板极电势，板极电位plate theory 薄板理论plateau 背脊platelet 薄片，小片platform 平台，站台plating 镀，电镀plating bath 镀金槽，电镀槽platinotron 泊管platinum (Pt)铂platinum black 铂黑platinum bolometer 铂测辐射热计platinum crucible 铂金坩埚platinum mirror coating 铂镜面镀层platinum oxide 氧化铂play (1)隙，隙缝(2)闪晃play-off beam 回扫射束playback 播收pleochroic 多向色的pleochroic halo 多向色晕圈pleochroism 多向色性，多色性Pleon lens 普来昂镜头plexiform layers 胶木板Plexiglass 普莱有机玻璃Plexiglass diffuser 普莱有机玻璃漫射体pliability 可挠性pliotron (1)功率三极管(2)空气过滤器pllane glass 平面玻璃，平板玻璃pllarising microscope in reflected light 反射光偏振微镜Plochere color system 普罗彻尔彩色系统Ploessl eyepiece 普罗爱瑟目镜，对称性目镜plot (1)标甥(2)标甥图plot diagram 点列图plotomat 自动绘图机plotter 标绘器，绘迹器plotting instrument 绘图仪器plotting objective 纠正仪物镜plotting tablet 标图板plug 插头，插塞plug gage 塞规plug screw gauge 螺纹塞规plug-in (1)捏入式的，组合式的(2)捏座plug-in unit 插件plugs 插头plumb 铅锤，垂直plumb aligner 铅锤对准器plumb level 水准仪，水平仪plumb line 铅垂线，重垂线plumb line deviation 垂线偏差plumbago 石墨，炭精plumbico camera tube 氧化铅光电导摄管plumbicon 氧化铅摄像管plumbing mirror 垂准镜plumbum (Pb)铅plummet (1)铅垂球(2)铅垂线plunger (1)柱塞(2)短路器plurality (1)多元(2)多数(3)复数plus (1)正号(2)加号(3)正数(4)正的(5)加plus lens 正透镜plus power spherical lens 正屈光度球透镜plus sphero-cylinder 正球柱透镜Plustar lens 普鲁斯特拉物镜plutonium (Pu)鐶PLZT display device PLZT显示装置PLZT wafers PLZT晶圆，钛酸锆酸铅晶圆PM-LCD Passive Matrix LCD被动式矩阵液晶PME-effect 光磁电效应pmmersion oil 浸油pneumatic 空气的，气动的，气力的，风力的pneumatic detector 气动检测器pneumatics 气体力学Pockels' cell 泡克耳斯拿Pockels' cell apodizer 泡克耳斯切趾器Pockels' device 泡克耳斯器件Pockels' effect 泡克耳斯效应Pockels' effect cell 泡克耳斯效应拿Pockels' readout optical nidulator (PROM)泡克耳斯读出光学调制器Pockels' shutter 泡克耳斯光闸pocket (1)袋，袋状物(2)袖珍的pocket camera 袖珍相机pocket compass 袖珍罗盘pocket microscope 袖珍显微镜pocket sextant 袖珍六分义Pohl interferometer 坡耳干涉仪Poincare sphere 鲍英卡勒球point (1)点(2)小数点(3)尖端point angle 顶角point brilliance 点耀度point caracteristic funtion 特微函数point defect 点缺陷point function 点函point object 点物point of fixation 注视点point of fusion 融汇点point of incidence 入射点point of inflexion 拐点，变曲点point of intersection 交点point of reference 参考点，基准点point particle 质点point projector microscope 点投射显微镜point sampling 点取样法point sighted 瞄准点point source 点光源point source lamp 点光源灯point source radiator 点源辐射器point spectrum 点谱，离散谱point spread function 点扩散函数point symetry 点对point symmetry groups 点对群point-by-point reproduction of the object 逐点再现物体point-by-point scanning 逐点扫描point-by-point storage 逐位存储point-contact photodiode 触光电二极管point-foussed 聚於一点的point-source hologram 点光源全息图point-to-point image 成点pointed 尖的pointer (1)指针(2)指示器pointing (1)削尖，弄尖(2)瞄准pointing accuracy 瞄准精度pointing error 瞄准误差pointing technique 瞄准技术pointolite 点光产pointolite lamp 点光源灯points of the compass 罗盘上方位罗Poisson diffraction 泊松衍射Poisson distribution 泊松分布Poisson's ratio 泊松比polanret microscope 变偏光相差显微镜polar angle 极角polar axis 极轴polar axis shaft 极坐标轴polar bond 极性polar compound 极性混合物polar corrdinates 极性标polar crystal 极性晶体polar diagram 极性坐标图polar distribution 极角分布polar light 极光polar molecule 有极分子，极性分子polar mosaic 极性镶嵌polar plot 极线图polar symmetry axis 极性对轴polar telescope 北极管polar vector 极矢polarimeter 偏振光计，偏振光镜polarimeters 偏振计polarimetric 测定偏振的polarimetric element 测振元件polarimetry 旋光测定法，测偏振术polarisation interferometer 偏振干涉仪polariscope 旋光计，偏振镜polariscopes 偏振光镜polarising metallurgical microscope 偏振金相显微镜polarising microscope in transmitted light 镜射光偏振显微镜polariton 电磁激子polariton scattering spectrum 电磁激子散射谱polarity 极性polarity detector 信号极生探测器polarizability (1)极化性(2)极化率polarizability ellipsoid 极化性椭球体polarizable 可极化polarizaiton of laser output 激光输出偏振度polarization (1)偏振(2)标定polarization analyzer 检偏器，检偏镜polarization degeneracy 极化简并度polarization effect 偏振效应polarization ellipse 偏振椭圆polarization error 极化误仪，偏振误差polarization factor 偏振因数polarization filter 偏振滤光器，偏振镜polarization hologram 偏振全息照片polarization interference microscope 偏光干涉显微镜polarization interferometer 偏振干涉仪polarization junction laser 偏振结型激光器polarization microscope 偏光显微镜polarization modulation 偏振调制polarization of light 光偏振polarization of sky light 天光偏裁polarization optics 偏振光学polarization photmeter 偏振光度计polarization reversal 极化又射polarization rotation (1)偏振旋转(2)极化旋转polarization selectivity 偏振选择性polarization tensor 极化张量polarization vector 偏振矢量polarization-rectifier obejctive 偏振整流物镜polarization-resolving optics 偏振光学分离器polarization-rotated reflection 偏振转反射polarizational labelling 偏振标定polarizationally isotropic cavity 偏振各向同性腔polarized headlight 偏振前车灯polarized light 偏振光polarized light microscope 偏振光显微镜polarized light modulation 偏振光调制polarized line 振线polarized radiation 极性辐射polarized specrophotometer 偏振分光光度计polarized wave 偏振波polarized-light optical system 振光光学系统polarizer 偏光板polarizer-Kerr-cell combination 偏振镜-克尔盒组合polarizer/ phase shift layer 偏光板/相位差板polarizers 偏光镜polarizign coil 极化线圈polarizing 起偏振polarizing angle 起偏抚角polarizing color filter 偏振彩色滤光片polarizing electrode 极化电极polarizing eyepiece 起偏振目镜polarizing filter 偏振滤光，起偏滤光polarizing filters 偏光滤光镜polarizing glass 偏光镜，偏振目镜polarizing itnerferometer 偏振干涉仪polarizing microscope 偏光显微镜polarizing microscopes 偏光显微镜polarizing mirror 偏光反射镜polarizing optics 偏振光学装置polarizing plate 起偏振光片polarizing prism 偏振棱镜，起偏棱镜polarizing shearing itnerferometer 偏振剪切干涉仪polarizing spectrohotmeter 偏振分光光度计polarizing waveplate 偏光波板polarizse (1)偏振(2)极化polarogram 极谱polarographci wave 极谱波polarographic analysis 极谱分析法polarographic method 极谱法polarography 极谱法polaroid (1)偏振片(2)即显胶片Polaroid camera 波拉一步照相机polaroid film 即显胶片polaroid filter 人造偏振片滤光器polaroid foil 人造偏振箱polaroid glass 偏光镜，偏振目镜polaroid polarizer 偏振片，偏振镜polaroid sheet 偏振片polaroid vectorgarph (1)偏振立体镜(2)立体电影polaron 极化子polarotactic navigation 偏振光导航polarotaxis 趋偏光性polaxis 极化轴pole (1)极(2)电极(3)磁极(4)极点(5)棒，杆pole figure 极像图pole gap 极隙pole piece 极片pole strenght 磁极强度pole-change 极变换polgrogaph 极谱仪poling 成极，单畴化polish (1)抛光(2)抛光剂polisher 抛光机polishing block 抛光盘polishing compound 抛光剂polishing compounds 研磨化合物polishing damage 抛光损伤polishing machine 抛光机polishing material 抛光材料polishing pads and cloth 研磨衬垫及布polishing plastic 抛光塑料polishing powder 抛光粉polishing rouge 抛光红粉polishing tool 抛光模politure 抛光polka-dot method 圆点光栅法pollutant 污染物pollution 污染pollution control 污染控制poloidal field 角向场poloniqum (Po)钋polsihed-barrl-rod 抛光圆棒polsihing felt 抛光毡子polsihing lap 抛光盘poly-lens objectie 多透镜物镜poly-silicon TFT LCD 多晶矽TFT LCD 液晶面板polyalkyl methacrylate 聚甲基丙烯酸烷基酯polyallyl metacylate 聚甲基丙烯酸烯丙酯polyamide 聚酸胺polyatomic 多原子polyatomic chemical laser 多原子激光器polyatomic system 多原子系统polycarbonate 聚碳酸酯polychormism 多组元polychroism 多色polychromatic 多色的polychromatic field 多色场polychromatic light 多色光，多色灯polychromatic radiation 多色辐射polychromatic radiator 多色辐射器polychromatic source 多色光源polychromatic wave 多色波polychromatism 多色polychromator 多色仪polychrome 多色性polycomponent 多晶体polycrystal 多晶的polycrystalline 多晶区polycrystalline area 多晶硫族化物polycrystalline chalcogenide 多晶纤维光学波导polycrystalline fibre optical waveguide 多晶锭块polycrystalline ingot 多晶激光器polycrystalline lawer 多晶物质polycrystalline material 多晶体散射polycrystalline scattering 多晶半导体polycrystalline semiconductor 聚甲基丙烯酸环已酯polycyclohexyl methacrylate 聚邻本二甲酸二烯丙酯polydiallyl itaconate 聚衣康酸二甲酯polydisperse 多色散polydisperse aqueous aerosol 多色散水气悬体polydisperse particulate system 多色散粒子系统polyemid 配向膜polyester 聚酯polyester fiber 聚酯纤维polyester-styrene 聚酯本乙烯polyethylen 聚乙烯polyethylene dimethacrylate 聚乙烯二异丁烯polyfoam 泡洙塑料polyglas 本乙烯塑料polygon 多形，多角形polygonal 多边形的，多角形的polygonal mirror 多面镜，光学夕面体polygonal mirrors 多面镜polygonal prism 多边形棱镜polyhedral 多面的polyhedron 多面体polyisobutylene 聚异乙烯polymer 聚合物Polymer processing 高分子加工Polymer science 高分子材料polymeric thin film 聚合薄膜polymerization 聚合作用polymerization transition 聚合变化polymerized 聚合的polymerized diacthylene 聚合丁二炔polymethacrylate 聚甲基丙烯酸酯polymethacrylic acid 聚甲基丙烯酸polymethine 聚甲炔polymethine dye laser 聚甲炔染料激光器polymethine Q switch 聚甲炔Q开关polymethyl methacrylate 聚甲基Polymethylmethacrylate diagnostic contact lens 诊疗用PMMA角膜接触镜片PMMA diagnostic contact lens 诊疗用PMMA角膜接触镜片polymorphism 聚甲基丙炔酸甲酯polynomial 同质多晶型polyphase 多项式多相polyphase current 多相电流polyplanar 多晶平面polypropylene 聚丙烯polyriboadenylic acid 多核糖腺甘酸polysilicon 多晶硅polystyrene 聚本孔烯polystyrene diffusion broadening 聚本乙烯扩展宽polytetrafluoethane 聚四氟甲烷polytetrafluorethylene (PTFE)聚四氟乙烯POLYTHENE FILM 聚乙烯polytroic 多方的polytype 多型体polyurethane 聚氨基甲酸醴polyvalence 多价polyvinyl 聚孔烯polyvinyl alcohol 聚孔烯醇polyvinyl chloride (PVC)聚氯孔烯polyvinyl chloride film 聚氯孔烯软片polyvinyl naphthalene 聚乙烯基本polyvinyl-fluoride 聚氟乙烯polyvinylcyclohexene dioxide 聚孔烯环已烯二氧化物polyvinylidene fluoride 聚偏氟乙烯ponderability 可称性，有质性pony axle 空转轴，导轴poop 尖锐脉冲poor focus 不银聚焦poor light condition 微光条件popular inversion area 粒子数反转区populated 粒子数增加的population (1)布居(2)粒子数population density 粒子数密度population difference 布居差population distribution 粒子数分布population hang-up 粒子数悬布population inversion 粒子数反转population mean 总体平均值population of level 能级个数，能级填满数population of parameter 参数组population ratio 粒子数比population risetime 粒子数增长时间population threshold 粒子数阈值porcelain 瓷器porcelain enamel 搪瓷porcelain insulator 瓷绝绿体porch 边缘pore 细孔pore diameter 孔径pore structure 细孔结构，毛孔结构porime focus 主反射镜焦点poriness 多孔性porosint 多孔材料porosity (1)多孔性(2)孔隙率porosu solid 多孔固体porous 多孔的porous cladding 多孔包层porous glass 多孔玻璃porous material 疏松材料porphyrin 咐林Porro prism 波罗棱镜Porro prism erecting system 波罗镜正像系统Porrotelescope 波罗望远镜。

少数载流子瞬态光谱(mcts)

少数载流子瞬态光谱(MCTS)是一种用于研究半导体材料中载流子动力学特性的先进技术。

通过测量材料中载流子的寿命和迁移率等参数，可以深入了解材料的电学性质，对半导体材料的研究和应用具有重要意义。

1. MCTS的原理和方法少数载流子瞬态光谱是使用激光脉冲来激发半导体材料，然后通过光电探测器测量样品的光学响应。

在这个过程中，材料中的载流子会发生非平衡状态的激发和复合过程，这些过程会导致材料的光学性质发生变化。

通过对这些变化的测量和分析，可以得到材料中载流子的寿命、迁移率等重要参数。

MCTS通常包括时间分辨和频率分辨两种方法。

时间分辨MCTS主要通过测量载流子在光激发后的动力学过程来研究载流子的特性，而频率分辨MCTS则通过对不同频率激发光的响应来获得频率依赖的载流子动力学信息。

2. MCTS在半导体材料研究中的应用MCTS在半导体材料研究中具有广泛的应用价值。

它可以帮助研究人员深入了解材料的电学性质，包括载流子的寿命、迁移率、复合过程等。

这些参数对于半导体材料的电子器件性能具有重要影响，因此MCTS 可以为新材料的研发和性能优化提供重要参考。

MCTS还可以用于研究半导体材料中的缺陷和杂质。

通过分析载流子动力学的变化，可以推断出材料中的缺陷类型和浓度等信息，这对于材料的质量控制和改进具有重要意义。

另外，MCTS也可以用于研究半导体材料中的光学特性和光电器件的工作原理。

通过对载流子动力学的研究，可以更好地理解材料的光吸收、发光等过程，为新型光电器件的设计和优化提供重要参考。

3. MCTS的发展和未来随着半导体材料和器件的不断发展，MCTS技术也在不断完善和拓展。

近年来，一些新型的光子学和超快光学技术被应用到MCTS中，如二维电子谱学、高阶谐波产生等，使得 MCTS 在时间和频率分辨能力上有了新的突破。

这些进展为更深入地研究和理解半导体材料的载流子动力学提供了新的途径，也为半导体光电器件的性能优化提供了更多可能性。

Two photon ionization of condensate atoms

a r X i v :p h y s i c s /0505055v 1 [p h y s i c s .a t o m -p h ] 8 M a y 2005Two photon ionization of condensate atomsM.Anderlini ∗and E.ArimondoINFM,Dipartimento di Fisica E.Fermi,Universit`a di Pisa,Largo Pontecorvo 3,I-56127Pisa,Italy(Dated:February 2,2008)The eﬃcient photoionization of a Bose-Einstein condensate requires the creation of ions with thesmallest possible transfer of atoms from the condensate into the thermal phase.The spontaneousdecay from excited states into atomic states with momentum diﬀerent from the initial one shouldbe reduced.We investigate theoretically the two-photon ionization of a rubidium condensate usingnear resonant excitation to the 6P state and second photon at 421nm or 1002nm into the contin-uum.Diﬀerent ionization schemes with coherent control of the ﬁrst excitation and reduction of thespontaneous decay are presented.PACS numbers:03.75.Nt,32.80.Rm,42.50.HzI.INTRODUCTION The realization of Bose-Einstein condensates (BEC)of alkali atom vapors has attracted much interest into new aspects of photon-matter interaction arising from the quantum nature of the atomic sample.Recently attention has been paid to the photoionization of a BEC by monochromatic laser light [1,2,3,4,5].The products of the pho-toionization process (electrons and ions)obey to Fermi-Dirac statistics.Due to the quantum nature of the initial atomic target and the narrow spectral width of the laser ionization source,the occupation number of the electron/ion ﬁnal states may become close to unity,especially for a laser excitation close to threshold.If this regime is reached the ionization rate could be slowed down by the Pauli blockade,with the occupation of ionized states determined by the balance between the laser ionization of the condensate and the rate of escape of the ionization products from the condensed system.Moreover,the electrostatic interaction between the ions and neutral atoms in the condensate is expected to originate localized deformations in the condensate density distribution.Either an increase or a decrease in the neutral atom spatial density,depending on the sign of the atom-ion phase shift,could be originated in small regions surrounding the ions [6].The experimental study of these processes requires the production of a large ionic concentration within a conden-sate on time shorter than the timescale on which the ions escape from the system,of the order of hundred ns for typical condensate samples,without simultaneously destroying the condensate.Instead,in the two-photon ionization experiments reported in refs.[2,3,4,5]the photoionization laser produced a signiﬁcant transfer of atoms from the condensate into the thermal cloud.Those experimental investigations demonstrated that the rubidium two-photon ionization was not performed in eﬃcient way,i.e.without producing a large depletion of the condensate atoms with their transformation into thermal atoms.Indeed,in typical two-photon ionization experiments the ionization is in-creased only at the expenses of a large production of thermal atoms.The present work reports a theoretical investigation of the eﬃciency realized in the ionization of atomic rubidium.This work addresses a target which is diﬀerent from those associated with the typical investigations of multiphoton processes.Our aim is not to increase the multiphoton ionization probability per se.In contrast,the production of ions is compared to optical pumping rate,produced by the spontaneous emission,transferring condensate atoms into the thermal cloud.The ionization eﬃciency will be determined by the decrease in the loss towards atomic states initially not occupied,i.e.a decrease of optical pumping into sink thermal states,and by the increase in the number of ions.Our condensate ionization scheme takes place in a three-level cascade scheme,the ﬁnal one being the atomic contin-uum.The condensate losses are produced by spontaneous emission from the intermediate excited state.An eﬃcient ionization is realized for an atomic transfer into the continuum with a reduced real excitation of the intermediate level,whence a reduced role of spontaneous emission processes from that intermediate level.The task of reducing the transfer towards sink levels is usually reached applying the STIRAP technique for the coherent transfer between the initial and ﬁnal levels of a three level scheme,as analyzed in [7,8],and for transfers to and from a continuum as in[9,10,11].An eﬃcient STIRAP transfer,which relies on the coherent coupling of the initial and the ﬁnal state,can be realized only using very short laser pulses,i. e.shorter than the characteristic decoherence time of the system.In the case of photoionization,the use of laser pulses shorter than the typical electron-ion decoherence times would prevent from creating the narrow kinetic energy distribution for the electrons and ions required for the Pauli blockade.Instead we will analyze here the two-photon ionization produced by narrow band cw radiation.For long interaction times the photoionization and optical pumping processes can be described through a simple rate equation approach.At shorter interaction times,comparable to or smaller than the spontaneous emission life-time from the ﬁrst excited state,the determination of the photoionization eﬃciency requires the solution of density matrix equations.Our solution of thedensity matrix equations searching for a regime of eﬃcient ionization has strong connections with the investigations of refs.[12,13]for the lineshapes of atomic excitation and ionization in a cascade three-level system under two-color excitation as a function of the time delay in the application of the two lasers.Section II describes the characteristics of our three-level scheme.Section III analyzes the case of simultaneous application of the excitation and photoionization lasers for long interaction times on the basis of the rate equation solution.In Section IV we analyze the ionization eﬃciency for diﬀerent temporal shapes of the excitation and ioniza-tion laser pulses using the density matrix approach.The lengths of the laser pulses and their time delay represent the experimental parameters controlling the ionization eﬃciency.Section V analyzes ionization based on a rapid adiabatic transfer.FIG.1:Three-level scheme for the excitation from the condensate ground state to the condensate ionic state through an intermediate |e state.The thermal sink state |s is occupied by the spontaneous emission decay from the |e state.II.ATOMIC LEVEL AND HAMILTONIANWe investigate the rubidium two-photon ionization scheme from the 5S 1/2ground state to the continuum with intermediate resonant state the 6P 3/2state at 2.94eV above the 5S 1/2ground state as in the experiment of ref.[4].The two-photon ionization was produced either by two photons a 421nm or by a two color process,one photon at 421nm and a second one at 1002nm.The photoionization cross sections for absorption of one 421nm or one 1002nm photon from the 6P state have a small diﬀerence,not playing an important role for the present investigation of the photoionization eﬃciency.Instead a larger intensity was available at the 1002nm wavelength,and the contribution to ionization of the two color process was much larger than the contribution of the one-color photoionization by two 421nm photons.The level scheme of Fig.1schematizes the two-photon ionization process from the ground state |g of the condensate to the condensate ionic state |i with a near resonant excitation of the intermediate level |e .The population of the intermediate state |e may decay back into the ground state through emission of spontaneous emission photons.For a sample composed by a Bose-Einstein condensate with atoms having a momentum p =0,the spontaneous emission produces ground state atoms with an atomic momentum p diﬀerent from zero.These atoms in the p =0ground state constitute a thermal component separate from the condensate,to be described through a sink state |s whose atomic properties are equivalent to those of the |g state.The |s state is ﬁlled by the spontaneous emission with rate Γfrom the |e state.The interaction of the |s state with the photoionization radiation is the same one of the |g state.However the photoionization from the |s state produces ions having an initial momentum p =0and weakly interacting with the condensate.Therefore that photoionization process is not relevant for our target of electrons/ions with a small kinetic energy[3]and it will not be considered into our analysis.A laser with angular frequency ω1,Rabi frequency Ω1and detuning δ=ω1−ωeg acts on the |g →|e transition,while a laser with angular frequency ω2and intensity I 2acts on the |e →|i transition.The intensity of the ﬁrstρi i + ρs s 1.20.80.40.0Time (µs)ρe e 1.20.80.40.0Time (µs)FIG.2:Comparison between the pertubative solution of Eqs.(8)(solidlines)and thenumericalsolution of Eqs.(1-5)(dashed lines)for the laser pulse shape shown as dotted line in (a).In (a)fraction ρee ,in (b)sum of ionized fraction ρii and of sink-state fraction ρss .Laser parameters I 1=0.66Wcm −2,corresponding to Ω1/2π=5.0MHz,I 2=2.0×103Wcm −2.laser is I 1=¯h ωeg 2˜ρeg −iδ˜ρeg −Ω1τ0ρii (t )dt ,(6)where the time τis longer than the laser pulse length τin order to include also the spontaneous emission losses towards the sink state at the end of the laser-atom interaction.Notice that in this analysis a large (small)value of r corresponds to a low (high)ionization eﬃciency.For any temporal shape of the applied laser pulses,a key parameter for the photoionization eﬃciency is the laser detuning δfrom the |g →|e transition.For decreasing detunings the two-photoionization process becomes more eﬃcient owing to the large excitation of the intermediate |e state;at the same time,however,also the spontaneous emission becomes more eﬃcient.As a consequence,for decreasing detunings the loss rate towards the |s state usually increases faster than the ionization rate,resulting in the decrease of eﬃciency for the condensate atoms photoionization.III.LONG LASER PULSESDuring the simultaneous application of the two lasers,the atomic population decays from the excited state|e and is lost from the system with total rate equal toΓL=Γ+σ1I1+σ2I2.Therefore,at every time t the total population in the|g and in the|e states decays with a time dependent loss rate equal toΓLρee(t).In the case in whichΓ,σi I i≪Ω1 the ionized fraction of population and the fraction lost by spontaneous emission can be estimated by treating the losses as a perturbation of the steady state solution for the Eqs.(1-5)restricted to the[|g ,|e ]two-level system.This perturbative solution isρpert ee (t)=Ω212Ω21+Γ2L+4δ2(Γ+σ1I1+σ2I2)t (7)Supposing the two lasers applied for a timeτthe ionic and sink state occupations may be written asρii(τ)=(σ1I1+σ2I2) τ0ρpert ee(t)dt=σ1I1+σ2I2τL ,ρss(τ)=Γ τ0ρpert ee(t)dt=ΓτL .(8) where we have introduced the timescaleτL=2Ω21+Γ2L+4δ2Γ+σ1I1+σ2I2(9)Therefore all the population is lost from the three-level system if the durationτof the pulses is much longer thanτL. In this limiting case,with no atom remaining in the|g state,the eﬃciency of the process is given byr∞=limτ→∞ρss(τ)σ1I1+σ2I2.(11)For instance,at laser intensity I1=0.66W cm−2corresponding to a Rabi frequencyΩ1/(2π)=5MHz and laser intensity I2=2000W cm−2,the f parameter is equal to53.8.IV.PULSED EXCITATIONAs demonstrated in refs[12,13],under the interaction with coherent pulsed radiation the atomic excitation presents diﬀerent properties than in measurements performed under the cw excitation regime.In the regime of pulsed exci-tation,the time evolution of populations and coherences becomes very important.For calculating the ionization eﬃciency it is necessary to perform the numerical solution of the density matrix equations(1-5).In fact,it should be taken into account that the actual occupationρee of the excited state is characterized by Rabi oscillations with eﬀective Rabi frequencyΩeﬀ1=τ0(σ1I1+σ2I2)ρee(t)dt=f+ρee(τ)δ/2π (MHz)101010ρi i δ/2π (MHz)FIG.3:In (a)ionized fraction ρii and in (b),continuous line,parameter r of the eﬃciency ratio versus the blue laser detuning δcalculated through the numerical solution of the density matrix equations.The dashed line reports the limiting value given by the f parameter of ser parameters I 1=0.66Wcm −2,corresponding to Ω1/2π=5.0MHz,I 2=2.0×103Wcm −2,interaction time τ=0.2/µs.In general r >f and the maximum eﬃciency of the ionization process corresponds to the minimum value r ∞=f .The oscillating behavior of the ionization process appears also measuring the ionization as function of the detuning δ.Such a behavior is shown by the results of Fig.3where the ionized fraction ρii and the eﬃciency ratio r are plotted as a function of the laser detuning δfrom the |e excited state,at ﬁxed interaction time τ.Owing to the choice τ=2π/Ω1,ρee (τ)=0,so that r has a minimum (and the eﬃciency has a maximum)at zero detuning.At larger values of the detunings ρii decreases with oscillations,and ρee (τ)assumes values dependent on the eﬀective frequency of the Rabi oscillations.The role of the Rabi oscillations is more clear on the plot of Fig.3(b)for the eﬃciency ratio r .For ﬁxed pulse duration the oscillating time dependence of the excited state population with detuning-dependent eﬀective Rabi frequency Ωeﬀ1produces an oscillating dependence of the ionized and of the lost fractions,and thus also of the ionization eﬃciency,as a function of the detuning δ/2π.In particular,the large oscillation of the ionization eﬃciency is determined by the oscillating amount of population remaining in the excited state |e at the end of the laser pulse and decaying totally by spontaneous emission into the sink state |s .As a consequece,r assumes oscillating values which are always larger than the f value of Eq.(11),and can be equal to that value only for isolated detunings where the eﬀective Rabi frequency Ωeﬀ1produces a 2πpulse for the applied duration of the atom-laser interaction.The occupation of the excited state at the end of the laser pulse can be annulled in the regime deﬁned as coherent population return[12,13].For the coherent excitation of two-level atomic systems subjected to light pulses of duration τand detuning δfrom resonance,the dynamics of the atomic population is adiabatic if the detuning is signiﬁcantly larger than the Fourier width of the pulse,i.e.,if δ≫1/τ.In this case the dressed state occupied at the end of the excitation is the same one as before the excitation.For a smooth pulse at constant detuning,that state coincides with the atomic ground state,so that even if the excited state is populated during the application of the pulse,all the population is adiabatically brought back to the ground state at the end of the process.Therefore no population fraction remains in the excited state,ρee (τ)=0.This regime of coherent population return does not produces any loss into the sink state at the laser pulse end.Results showing the advantages of this regime in the two-photon ionization are reported in Fig.4.We considered several durations of pulses all of them with the shape shown in Fig.4(a).For each duration of the IR square pulse,the blue intensity had a Gaussian proﬁle such that the intensity decreased to 1/e 3of the peak value in correspondence of the IR pulse start and end.In Fig.4(b)and (c)the ionized fraction ρii and the parameter r of the the ratio between the atomic fraction in the sink state and the ionized fraction are shown,respectively,as a function of the laser detuning δ,for several durations of the laser pulse and at a given blue Rabi frequency.In all cases,the eﬀects of adiabatic excitation are visible,because for each pulse duration there is a minimum detuning necessary to obtain the optimal ratio r .The value of the minimum detuning is only slightly sensitive to the Rabi frequency of the transition,because,as pointed out in [12],no power broadening occurs in a scheme of coherent population return.The limiting value of r reached at large detunings corresponds to the minimum value f calculated at the laser intensitiesδ1/(2 π) (MHz)10-810-710-610-510-410-3 δ1/(2 π) (MHz) N o r m a l i z e d I n t e n s i t y FIG.4:Ionization with coherent population return.In a)temporal shapes of the blue/IR laser for a 125ns time length of the IR pulse.In b)ionic occupation ρii and in c)parameter r describing the eﬃciency ratio versus laser detuning δ/2π,in MHz.Ω1/2π=5.MHz,I 2=1500W/cm 2,f =71.7.Results for diﬀerent time lengths of the IR pulse,30,60,125and 250ns respectively,with the blue pulse length scaled as shown in a)and discussed in the text.of the numerical analysis.V.RAPID ADIABATIC PASSAGEAnother excitation scheme allowing an eﬃcient and robust transfer of the population from the ground to the excited state is the rapid adiabatic passage (RAP)technique[17,18].This technique takes advantage of the population adiabatic evolution in order to transfer almost the whole population from the ground state to the excited state,blocking completely the population return.RAP is produced by a time dependence of the laser detuning,and for an eﬃcient transfer the laser detuning changes from a very large and negative value to a very large and positive value,or vice versa,during the application of the light.The laser frequency experiences a chirp,and the chirp duration should be shorter than the excited state lifetime.Under these circumstances,the lowest energy adiabatic state of the system coincides with the ground state before the application of the light and with the excited state at the end of the process.When this scheme is applied to the lower atomic transition of the rubidium two-photon ionization,it allows to transfer the ground state population to the |6P state,from where a pulse of IR light,with the time sequence shown in Fig.5(left-hand side),produces the ionization shown in Fig.5(right-hand side).Because the IR pulse ionizes the excited state atoms and also modiﬁes the energy of the excited state,for any temporal shape of the two laser pulses the largest two-photon ionization is obtained for a particular delay of the IR pulse with respect to the blue laser pulse.For the pulse shapes of Fig.5the maximum ionization was obtained when the center of the IR pulse was delayed by 0.02µs with respect to the center of the blue pulse.A linear chirp of the blue detuning was examined,but the chirp exact shape had a minor inﬂuence on the ionization eﬃciency,provided that the detuning diﬀerence between initial and ﬁnal value was larger than ∼100MHz.Suppressing the oscillatory behavior of the excited population,the RAP led to an excited state occupation and an ionized fraction larger than those obtained by pulses of the same shape but at constant blue detuning.For the blue laser scanning of Fig.5the ionization fraction was larger by a factor 2than produced at constant blue detuning (0.0057against 0.0027).The RAP technique is thus suitable for producing the maximum possible fraction of ionized population within a laser pulse sequence of short duration,during which the ratio between the atomic population in the sink state and the atomic population in the ionic state corresponds toTime (µs)FIG.5:Ionization using RAP from the ground state to the excited state.In left plot the temporal shapes of the blue(dotted line)and IR(dashed line)laser pulses and of the blue laser detuning(continuous line)δ/2π,in MHz,atΩ1/2π=30.7MHz and I2=2000W/cm−2.In right plot time evolution of the excited state occupation(solid line)and of ionized fraction excited state(dashed line).the optimal value f.Due to the suppression of the coherent population return,on the other hand,a large fraction of population typically remains in the excited state at the end of the laser pulse and decays into the sink state thereafter. As a consequence,in RAP at long interaction times the eﬃciency parameter r,calculated according to Eq.6,reaches values larger than those associated to the adiabatic regime.For the parameters considered above the eﬃciency ratio r was165against the minimum value f=53.8.VI.CONCLUSIONWe have examined a problem to be classiﬁed as a quantum control one:reach a speciﬁc target,the ionization of the condensate,minimizing the losses into a diﬀerent output channel,the production of thermal atoms.We have explored diﬀerent two-photon ionization schemes,producing diﬀerent quantum controls for the atomic excited state. However the quantum control reached for the two-photon ionization is very poor.In eﬀect the ionization process transferring atoms from the excited state to the ionization state is governed by rate equations,whence a classical response instead of a quantum one.Ourﬁnal result is that the maximum ionization eﬃciency to be reached through the quantum control on theﬁrst excitation,depends on the probabilities of two processes,the spontaneous decay rate and the ionization rate.These rate are not modiﬁed within the quantum control of our analysis.While the qualitative behavior of the ionized fraction depends only on the properties of the laser pulse exciting theﬁrst step of the ionization sequence,the absolute value of the ionized fraction depends only on the intensity of the IR light coupling the excited state to the continuum.An analogous statement applies also to the eﬃciency ratio between the thermal fraction and the ionized fraction.The criteria we have derived for the optimization of the two-photon ionization have a general validity,whereas the absolute numbers we have discussed correspond to the intensities available in the analyzed experiment.The fraction of ionized atoms in these conditions is much smaller than the number of photons scattered by each atom.The application of the optimization criteria we have derived, however,together with IR intensities larger than those we have considered,will allow to reach experimental conditions leading to an eﬃcient large photoionization of Bose-Einstein condensates.VII.ACKNOWLEDGEMENTSThis research was supported by the INFM-Italy through the PhotonMatter Project,by the MIUR-Italy through a PRIN Project,and by the European Commission through the Cold Quantum-Gases Network,contract HPRN-CT-2000-00125.E.A.thanks N.Vitanov for useful discussions.[1]I.E.Mazets,Quantum Semiclass.Opt.10,675-681(1999).[2]D.Ciampini,M.Anderlini,J.H.M¨u ller,F.Fuso,O.Morsch,J.W.Thomsen,and E.Arimondo,Phys.Rev.A66,043409(2002).[3]M.Anderlini,D.Ciampini,E.Courtade,F.Fuso,O.Morsch,J.H.M¨u ller and E.Arimondo,in”Laser Spectroscopy”,Proceedings of the XVI International Conference,eds.P.Hannaford,A.Sidorov,H.Bachor and K.Baldwin,(World Scientiﬁc,2004)p.353-361.[4]M.Anderlini,E.Courtade,M.Anderlini,D.Ciampini,J.H.M¨u ller,O.Morsch,and E.Arimondo,J.Opt.Soc.Amer.B21,840-5(2004).[5]E.Courtade,M.Anderlini,D.Ciampini,J.H.M¨u ller,O.Morsch,E.Arimondo,M.Aymar,and E.J.Robinson,J.Phys.B:At.Mol.Opt.Phys.37,967-979(2004).[6]P.Massignan,C.J.Pethick,and H.Smith,Phys.Rev.A71,023606(2005).[7]K.Bergmann,H.Theuer,and B.W.Shore,Rev.Mod.Phys.70,1003-1025(1998).[8]N.V.Vitanov,T.Halfmann,B.W.Shore and K.Bergmann,Ann.Rev.Phys.Chem.52,763-809(2001).[9]A.Vardi,M.Shapiro,and K.Bergmann,Optics Express4,91(1999).[10]M.Mackie,R.Kowalski,and J.Javanainen,Phys.Rev.Lett.84,3803-6(2000)and reference therein.[11]A.Vardi,M.Shapiro,and J.R.Anglin,Phys.Rev.A65,027401(2002).[12]N.V.Vitanov,B.W.Shore,L.Yatsenko,K.B¨o hmer,T.Halfmann,T.Rickes and K.Bergmann,m.199,117-126(2001).[13]T.Halfmann,T.Rickes,N.V.Vitanov,and K.Bergmann,m.220,353-359(2003).[14]mbropoulos,Phys.Rev.A9,1992-2013(1974).[15]B.W.Shore,The Theory of Coherent Atomic Excitation,vols.1and2(John Wiley,New York,1990).[16]A.Adler,A.Rachman,and E.J.Robinson,J.Phys.B:At.Mol.Opt.Phys.28,5057-5075(1995).[17]A.Abragam,The principles of nuclear magnetism,(Clarendon,Oxford,1961).[18]S.Gu´e rin,S.Thomas,and H.R.Jauslin,Phys.Rev.A65,023409(2002).。

裴攀-翻译中文

第6章光源和放大器在光纤系统，光纤光源产生的光束携带的信息。

激光二极管和发光二极管是两种最常见的来源。

他们的微小尺寸与小直径的光纤兼容，其坚固的结构和低功耗要求与现代的固态电子兼容。

在以下几个GHz的工作系统，大部分（或数Gb /秒），信息贴到光束通过调节输入电流源。

外部调制（在第4、10章讨论）被认为是当这些率超标。

我们二极管LED和激光研究，包括操作方法，转移特性和调制。

我们计划以获得其他好的或理念的差异的两个来源，什么情况下调用。

当纤维损失导致信号功率低于要求的水平，光放大器都需要增强信号到有效的水平。

通过他们的使用，光纤链路可以延长。

因为光源和光放大器，如此多的共同点，他们都是在这一章处理。

1.发光二极管一个发光二极管[1，2]是一个PN结的半导体发光时正向偏置。

图6.1显示的连接器件、电路符号，能量块和二极管关联。

能带理论提供了对一个）简单的解释半导体发射器（和探测器）。

允许能带通过的是工作组，其显示的宽度能在图中，相隔一禁止区域（带隙）。

在上层能带称为导带，电子不一定要到移动单个原子都是免费的。

洞中有一个正电荷。

它们存在于原子电子的地点已经从一个中立带走，留下的电荷原子与净正。

自由电子与空穴重新结合可以，返回的中性原子状态。

能量被释放时，发生这种情况。

一个n -型半导体拥有自由电子数，如图图英寸6.1。

p型半导体有孔数自由。

当一种P型和一种N型材料费米能级（WF）的P和N的材料一致，并外加电压上作用时，产生的能垒如显示的数字所示。

重参杂材料，这种情况提供许多电子传到和过程中需要排放的孔。

在图中，电子能量增加垂直向上，能增加洞垂直向下。

因此，在N地区的自由电子没有足够的能量去穿越阻碍而移动到P区。

同样，空穴缺乏足够的能量克服障碍而移动进入n区。

当没有外加电压时，由于两种材料不同的费米能级产生的的能量阻碍，就不能自由移动。

外加电压通过升高的N端势能，降低一侧的P端势能，从而是阻碍减小。

如果供电电压（电子伏特）与能级（工作组）相同，自由电子和自由空穴就有足够的能量移动到交界区，如底部的数字显示，当一个自由电子在交界区遇到了一个空穴，电子可以下降到价带，并与空穴重组。

电子信息工程专业英语翻译清华出版社EnglishforITandEE03

will experience a force (in a similar way that planets
experience a force in the gravitational field of the Sun). If
电子信息工程专业英语翻译清华出版社
EnglishforITandEE03
2020/11/27
电子信息工程专业英语翻译清华出版社EnglishforITandEE03
Unit 3
EM Fields, Antenna and Microwaves
电子信息工程专业英语翻译清华出版社EnglishforITandEE03
two locations. For instance, the metal atoms in a radio
transmitter appear to transfer energy continuously. This view
is useful to a certain extent (radiation of low frequency), but
Part I
Electromagnetic Field
电子信息工程专业英语翻译清华出版社EnglishforITandEE03
New Words
vicinity 邻近，附近 charge 电荷 photon 光子 atom 原子 catastrophe 大灾难，大祸 emission 发射，散发 incident 入射的 electrodynamics 电动力学 gravitational 重力的
•随着时间的推移，人们认识到电场和磁场是电磁场这一整体的两个部分。
电子信息工程专业英语翻译清华出版社EnglishforITandEE03

Principles of Plasma Discharges and Materials Processing9

CHAPTER8MOLECULAR COLLISIONS8.1INTRODUCTIONBasic concepts of gas-phase collisions were introduced in Chapter3,where we described only those processes needed to model the simplest noble gas discharges: electron–atom ionization,excitation,and elastic scattering;and ion–atom elastic scattering and resonant charge transfer.In this chapter we introduce other collisional processes that are central to the description of chemically reactive discharges.These include the dissociation of molecules,the generation and destruction of negative ions,and gas-phase chemical reactions.Whereas the cross sections have been measured reasonably well for the noble gases,with measurements in reasonable agreement with theory,this is not the case for collisions in molecular gases.Hundreds of potentially signiﬁcant collisional reactions must be examined in simple diatomic gas discharges such as oxygen.For feedstocks such as CF4/O2,SiH4/O2,etc.,the complexity can be overwhelming.Furthermore,even when the signiﬁcant processes have been identiﬁed,most of the cross sections have been neither measured nor calculated. Hence,one must often rely on estimates based on semiempirical or semiclassical methods,or on measurements made on molecules analogous to those of interest. As might be expected,data are most readily available for simple diatomic and polyatomic gases.Principles of Plasma Discharges and Materials Processing,by M.A.Lieberman and A.J.Lichtenberg. ISBN0-471-72001-1Copyright#2005John Wiley&Sons,Inc.235236MOLECULAR COLLISIONS8.2MOLECULAR STRUCTUREThe energy levels for the electronic states of a single atom were described in Chapter3.The energy levels of molecules are more complicated for two reasons. First,molecules have additional vibrational and rotational degrees of freedom due to the motions of their nuclei,with corresponding quantized energies E v and E J. Second,the energy E e of each electronic state depends on the instantaneous con-ﬁguration of the nuclei.For a diatomic molecule,E e depends on a single coordinate R,the spacing between the two nuclei.Since the nuclear motions are slow compared to the electronic motions,the electronic state can be determined for anyﬁxed spacing.We can therefore represent each quantized electronic level for a frozen set of nuclear positions as a graph of E e versus R,as shown in Figure8.1.For a mole-cule to be stable,the ground(minimum energy)electronic state must have a minimum at some value R1corresponding to the mean intermolecular separation (curve1).In this case,energy must be supplied in order to separate the atoms (R!1).An excited electronic state can either have a minimum( R2for curve2) or not(curve3).Note that R2and R1do not generally coincide.As for atoms, excited states may be short lived(unstable to electric dipole radiation)or may be metastable.Various electronic levels may tend to the same energy in the unbound (R!1)limit. Array FIGURE8.1.Potential energy curves for the electronic states of a diatomic molecule.For diatomic molecules,the electronic states are speciﬁedﬁrst by the component (in units of hÀ)L of the total orbital angular momentum along the internuclear axis, with the symbols S,P,D,and F corresponding to L¼0,+1,+2,and+3,in analogy with atomic nomenclature.All but the S states are doubly degenerate in L.For S states,þandÀsuperscripts are often used to denote whether the wave function is symmetric or antisymmetric with respect to reﬂection at any plane through the internuclear axis.The total electron spin angular momentum S (in units of hÀ)is also speciﬁed,with the multiplicity2Sþ1written as a preﬁxed superscript,as for atomic states.Finally,for homonuclear molecules(H2,N2,O2, etc.)the subscripts g or u are written to denote whether the wave function is sym-metric or antisymmetric with respect to interchange of the nuclei.In this notation, the ground states of H2and N2are both singlets,1Sþg,and that of O2is a triplet,3SÀg .For polyatomic molecules,the electronic energy levels depend on more thanone nuclear coordinate,so Figure8.1must be generalized.Furthermore,since there is generally no axis of symmetry,the states cannot be characterized by the quantum number L,and other naming conventions are used.Such states are often speciﬁed empirically through characterization of measured optical emission spectra.Typical spacings of low-lying electronic energy levels range from a few to tens of volts,as for atoms.Vibrational and Rotational MotionsUnfreezing the nuclear vibrational and rotational motions leads to additional quan-tized structure on smaller energy scales,as illustrated in Figure8.2.The simplest (harmonic oscillator)model for the vibration of diatomic molecules leads to equally spaced quantized,nondegenerate energy levelse E v¼hÀv vib vþ1 2(8:2:1)where v¼0,1,2,...is the vibrational quantum number and v vib is the linearized vibration frequency.Fitting a quadratic functione E v¼12k vib(RÀ R)2(8:2:2)near the minimum of a stable energy level curve such as those shown in Figure8.1, we can estimatev vib%k vibm Rmol1=2(8:2:3)where k vib is the“spring constant”and m Rmol is the reduced mass of the AB molecule.The spacing hÀv vib between vibrational energy levels for a low-lying8.2MOLECULAR STRUCTURE237stable electronic state is typically a few tenths of a volt.Hence for molecules in equi-librium at room temperature (0.026V),only the v ¼0level is signiﬁcantly popula-ted.However,collisional processes can excite strongly nonequilibrium vibrational energy levels.We indicate by the short horizontal line segments in Figure 8.1a few of the vibrational energy levels for the stable electronic states.The length of each segment gives the range of classically allowed vibrational motions.Note that even the ground state (v ¼0)has a ﬁnite width D R 1as shown,because from(8.2.1),the v ¼0state has a nonzero vibrational energy 1h Àv vib .The actual separ-ation D R about Rfor the ground state has a Gaussian distribution,and tends toward a distribution peaked at the classical turning points for the vibrational motion as v !1.The vibrational motion becomes anharmonic and the level spa-cings tend to zero as the unbound vibrational energy is approached (E v !D E 1).FIGURE 8.2.Vibrational and rotational levels of two electronic states A and B of a molecule;the three double arrows indicate examples of transitions in the pure rotation spectrum,the rotation–vibration spectrum,and the electronic spectrum (after Herzberg,1971).238MOLECULAR COLLISIONSFor E v.D E1,the vibrational states form a continuum,corresponding to unbound classical motion of the nuclei(breakup of the molecule).For a polyatomic molecule there are many degrees of freedom for vibrational motion,leading to a very compli-cated structure for the vibrational levels.The simplest(dumbbell)model for the rotation of diatomic molecules leads to the nonuniform quantized energy levelse E J¼hÀ22I molJ(Jþ1)(8:2:4)where I mol¼m Rmol R2is the moment of inertia and J¼0,1,2,...is the rotational quantum number.The levels are degenerate,with2Jþ1states for the J th level. The spacing between rotational levels increases with J(see Figure8.2).The spacing between the lowest(J¼0to J¼1)levels typically corresponds to an energy of0.001–0.01V;hence,many low-lying levels are populated in thermal equilibrium at room temperature.Optical EmissionAn excited molecular state can decay to a lower energy state by emission of a photon or by breakup of the molecule.As shown in Figure8.2,the radiation can be emitted by a transition between electronic levels,between vibrational levels of the same electronic state,or between rotational levels of the same electronic and vibrational state;the radiation typically lies within the optical,infrared,or microwave frequency range,respectively.Electric dipole radiation is the strongest mechanism for photon emission,having typical transition times of t rad 10À9s,as obtained in (3.4.13).The selection rules for electric dipole radiation areDL¼0,+1(8:2:5a)D S¼0(8:2:5b) In addition,for transitions between S states the only allowed transitions areSþÀ!Sþand SÀÀ!SÀ(8:2:6) and for homonuclear molecules,the only allowed transitions aregÀ!u and uÀ!g(8:2:7) Hence homonuclear diatomic molecules do not have a pure vibrational or rotational spectrum.Radiative transitions between electronic levels having many different vibrational and rotational initial andﬁnal states give rise to a structure of emission and absorption bands within which a set of closely spaced frequencies appear.These give rise to characteristic molecular emission and absorption bands when observed8.2MOLECULAR STRUCTURE239using low-resolution optical spectrometers.As for atoms,metastable molecular states having no electric dipole transitions to lower levels also exist.These have life-times much exceeding10À6s;they can give rise to weak optical band structures due to magnetic dipole or electric quadrupole radiation.Electric dipole radiation between vibrational levels of the same electronic state is permitted for molecules having permanent dipole moments.In the harmonic oscillator approximation,the selection rule is D v¼+1;weaker transitions D v¼+2,+3,...are permitted for anharmonic vibrational motion.The preceding description of molecular structure applies to molecules having arbi-trary electronic charge.This includes neutral molecules AB,positive molecular ions ABþ,AB2þ,etc.and negative molecular ions ABÀ.The potential energy curves for the various electronic states,regardless of molecular charge,are commonly plotted on the same diagram.Figures8.3and8.4give these for some important electronic statesof HÀ2,H2,and Hþ2,and of OÀ2,O2,and Oþ2,respectively.Examples of both attractive(having a potential energy minimum)and repulsive(having no minimum)states can be seen.The vibrational levels are labeled with the quantum number v for the attrac-tive levels.The ground states of both Hþ2and Oþ2are attractive;hence these molecular ions are stable against autodissociation(ABþ!AþBþor AþþB).Similarly,the ground states of H2and O2are attractive and lie below those of Hþ2and Oþ2;hence they are stable against autodissociation and autoionization(AB!ABþþe).For some molecules,for example,diatomic argon,the ABþion is stable but the AB neutral is not stable.For all molecules,the AB ground state lies below the ABþground state and is stable against autoionization.Excited states can be attractive or repulsive.A few of the attractive states may be metastable;some examples are the 3P u state of H2and the1D g,1Sþgand3D u states of O2.Negative IonsRecall from Section7.2that many neutral atoms have a positive electron afﬁnity E aff;that is,the reactionAþeÀ!AÀis exothermic with energy E aff(in volts).If E aff is negative,then AÀis unstable to autodetachment,AÀ!Aþe.A similar phenomenon is found for negative molecular ions.A stable ABÀion exists if its ground(lowest energy)state has a potential minimum that lies below the ground state of AB.This is generally true only for strongly electronegative gases having large electron afﬁnities,such as O2 (E aff%1:463V for O atoms)and the halogens(E aff.3V for the atoms).For example,Figure8.4shows that the2P g ground state of OÀ2is stable,with E aff% 0:43V for O2.For weakly electronegative or for electropositive gases,the minimum of the ground state of ABÀgenerally lies above the ground state of AB,and ABÀis unstable to autodetachment.An example is hydrogen,which is weakly electronegative(E aff%0:754V for H atoms).Figure8.3shows that the2Sþu ground state of HÀ2is unstable,although the HÀion itself is stable.In an elec-tropositive gas such as N2(E aff.0),both NÀ2and NÀare unstable. 240MOLECULAR COLLISIONS8.3ELECTRON COLLISIONS WITH MOLECULESThe interaction time for the collision of a typical (1–10V)electron with a molecule is short,t c 2a 0=v e 10À16–10À15s,compared to the typical time for a molecule to vibrate,t vib 10À14–10À13s.Hence for electron collisional excitation of a mole-cule to an excited electronic state,the new vibrational (and rotational)state canbeFIGURE 8.3.Potential energy curves for H À2,H 2,and H þ2.(From Jeffery I.Steinfeld,Molecules and Radiation:An Introduction to Modern Molecular Spectroscopy ,2d ed.#MIT Press,1985.)8.3ELECTRON COLLISIONS WITH MOLECULES 241FIGURE 8.4.Potential energy curves for O À2,O 2,and O þ2.(From Jeffery I.Steinfeld,Molecules and Radiation:An Introduction to Modern Molecular Spectroscopy ,2d ed.#MIT Press,1985.)242MOLECULAR COLLISIONS8.3ELECTRON COLLISIONS WITH MOLECULES243 determined by freezing the nuclear motions during the collision.This is known as the Franck–Condon principle and is illustrated in Figure8.1by the vertical line a,showing the collisional excitation atﬁxed R to a high quantum number bound vibrational state and by the vertical line b,showing excitation atﬁxed R to a vibra-tionally unbound state,in which breakup of the molecule is energetically permitted. Since the typical transition time for electric dipole radiation(t rad 10À9–10À8s)is long compared to the dissociation( vibrational)time t diss,excitation to an excited state will generally lead to dissociation when it is energetically permitted.Finally, we note that the time between collisions t c)t rad in typical low-pressure processing discharges.Summarizing the ordering of timescales for electron–molecule collisions,we havet at t c(t vib t diss(t rad(t cDissociationElectron impact dissociation,eþABÀ!AþBþeof feedstock gases plays a central role in the chemistry of low-pressure reactive discharges.The variety of possible dissociation processes is illustrated in Figure8.5.In collisions a or a0,the v¼0ground state of AB is excited to a repulsive state of AB.The required threshold energy E thr is E a for collision a and E a0for Array FIGURE8.5.Illustrating the variety of dissociation processes for electron collisions with molecules.collision a0,and it leads to an energy after dissociation lying between E aÀE diss and E a0ÀE diss that is shared among the dissociation products(here,A and B). Typically,E aÀE diss few volts;consequently,hot neutral fragments are typically generated by dissociation processes.If these hot fragments hit the substrate surface, they can profoundly affect the process chemistry.In collision b,the ground state AB is excited to an attractive state of AB at an energy E b that exceeds the binding energy E diss of the AB molecule,resulting in dissociation of AB with frag-ment energy E bÀE diss.In collision b0,the excitation energy E b0¼E diss,and the fragments have low energies;hence this process creates fragments having energies ranging from essentially thermal energies up to E bÀE diss few volts.In collision c,the AB atom is excited to the bound excited state ABÃ(labeled5),which sub-sequently radiates to the unbound AB state(labeled3),which then dissociates.The threshold energy required is large,and the fragments are hot.Collision c can also lead to dissociation of an excited state by a radiationless transfer from state5to state4near the point where the two states cross:ABÃðboundÞÀ!ABÃðunboundÞÀ!AþBÃThe fragments can be both hot and in excited states.We discuss such radiationless electronic transitions in the next section.This phenomenon is known as predisso-ciation.Finally,a collision(not labeled in theﬁgure)to state4can lead to dis-sociation of ABÃ,again resulting in hot excited fragments.The process of electron impact excitation of a molecule is similar to that of an atom,and,consequently,the cross sections have a similar form.A simple classical estimate of the dissociation cross section for a level having excitation energy U1can be found by requiring that an incident electron having energy W transfer an energy W L lying between U1and U2to a valence electron.Here,U2is the energy of the next higher level.Then integrating the differential cross section d s[given in(3.4.20)and repeated here],d s¼pe24021Wd W LW2L(3:4:20)over W L,we obtains diss¼0W,U1pe24pe021W1U1À1WU1,W,U2pe24021W1U1À1U2W.U28>>>>>><>>>>>>:(8:3:1)244MOLECULAR COLLISIONSLetting U2ÀU1(U1and introducing voltage units W¼e E,U1¼e E1and U2¼e E2,we haves diss¼0E,E1s0EÀE11E1,E,E2s0E2ÀE1EE.E28>>>><>>>>:(8:3:2)wheres0¼pe4pe0E12(8:3:3)We see that the dissociation cross section rises linearly from the threshold energy E thr%E1to a maximum value s0(E2ÀE1)=E thr at E2and then falls off as1=E. Actually,E1and E2can depend on the nuclear separation R.In this case,(8.3.2) should be averaged over the range of R s corresponding to the ground-state vibrational energy,leading to a broadened dependence of the average cross section on energy E.The maximum cross section is typically of order10À15cm2. Typical rate constants for a single dissociation process with E thr&T e have an Arrhenius formK diss/K diss0expÀE thr T e(8:3:4)where K diss0 10À7cm3=s.However,in some cases E thr.T e.For excitation to an attractive state,an appropriate average over the fraction of the ground-state vibration that leads to dissociation must be taken.Dissociative IonizationIn addition to normal ionization,eþABÀ!ABþþ2eelectron–molecule collisions can lead to dissociative ionizationeþABÀ!AþBþþ2eThese processes,common for polyatomic molecules,are illustrated in Figure8.6.In collision a having threshold energy E iz,the molecular ion ABþis formed.Collisionsb andc occur at higher threshold energies E diz and result in dissociative ionization,8.3ELECTRON COLLISIONS WITH MOLECULES245leading to the formation of fast,positively charged ions and neutrals.These cross sections have a similar form to the Thompson ionization cross section for atoms.Dissociative RecombinationThe electron collision,e þAB þÀ!A þB Ãillustrated as d and d 0in Figure 8.6,destroys an electron–ion pair and leads to the production of fast excited neutral fragments.Since the electron is captured,it is not available to carry away a part of the reaction energy.Consequently,the collision cross section has a resonant character,falling to very low values for E ,E d and E .E d 0.However,a large number of excited states A Ãand B Ãhaving increasing principal quantum numbers n and energies can be among the reaction products.Consequently,the rate constants can be large,of order 10À7–10À6cm 3=s.Dissocia-tive recombination to the ground states of A and B cannot occur because the potential energy curve for AB þis always greater than the potential energycurveFIGURE 8.6.Illustration of dissociative ionization and dissociative recombination for electron collisions with molecules.246MOLECULAR COLLISIONSfor the repulsive state of AB.Two-body recombination for atomic ions or for mol-ecular ions that do not subsequently dissociate can only occur with emission of a photon:eþAþÀ!Aþh n:As shown in Section9.2,the rate constants are typically three toﬁve orders of magnitude lower than for dissociative recombination.Example of HydrogenThe example of H2illustrates some of the inelastic electron collision phenomena we have discussed.In order of increasing electron impact energy,at a threshold energy of 8:8V,there is excitation to the repulsive3Sþu state followed by dissociation into two fast H fragments carrying 2:2V/atom.At11.5V,the1Sþu bound state is excited,with subsequent electric dipole radiation in the ultraviolet region to the1Sþg ground state.At11.8V,there is excitation to the3Sþg bound state,followedby electric dipole radiation to the3Sþu repulsive state,followed by dissociation with 2:2V/atom.At12.6V,the1P u bound state is excited,with UV emission tothe ground state.At15.4V,the2Sþg ground state of Hþ2is excited,leading to the pro-duction of Hþ2ions.At28V,excitation of the repulsive2Sþu state of Hþ2leads to thedissociative ionization of H2,with 5V each for the H and Hþfragments.Dissociative Electron AttachmentThe processes,eþABÀ!AþBÀproduce negative ion fragments as well as neutrals.They are important in discharges containing atoms having positive electron afﬁnities,not only because of the pro-duction of negative ions,but because the threshold energy for production of negative ion fragments is usually lower than for pure dissociation processes.A variety of pro-cesses are possible,as shown in Figure8.7.Since the impacting electron is captured and is not available to carry excess collision energy away,dissociative attachment is a resonant process that is important only within a narrow energy range.The maximum cross sections are generally much smaller than the hard-sphere cross section of the molecule.Attachment generally proceeds by collisional excitation from the ground AB state to a repulsive ABÀstate,which subsequently either auto-detaches or dissociates.The attachment cross section is determined by the balance between these processes.For most molecules,the dissociation energy E diss of AB is greater than the electron afﬁnity E affB of B,leading to the potential energy curves shown in Figure8.7a.In this case,the cross section is large only for impact energies lying between a minimum value E thr,for collision a,and a maximum value E0thr for8.3ELECTRON COLLISIONS WITH MOLECULES247FIGURE 8.7.Illustration of a variety of electron attachment processes for electron collisions with molecules:(a )capture into a repulsive state;(b )capture into an attractive state;(c )capture of slow electrons into a repulsive state;(d )polar dissociation.248MOLECULAR COLLISIONScollision a 0.The fragments are hot,having energies lying between minimum and maximum values E min ¼E thr þE affB ÀE diss and E max ¼E 0thr þE af fB ÀE diss .Since the AB Àstate lies above the AB state for R ,R x ,autodetachment can occur as the mol-ecules begin to separate:AB À!AB þe.Hence the cross section for production of negative ions can be much smaller than that for excitation of the AB Àrepulsive state.As a crude estimate,for the same energy,the autodetachment rate is ﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃﬃM R =m p 100times the dissociation rate of the repulsive AB Àmolecule,where M R is the reduced mass.Hence only one out of 100excitations lead to dissociative attachment.Excitation to the AB Àbound state can also lead to dissociative attachment,as shown in Figure 8.7b .Here the cross section is signiﬁcant only for E thr ,E ,E 0thr ,but the fragments can have low energies,with a minimum energy of zero and a maximum energy of E 0thr þE affB ÀE diss .Collision b,e þAB À!AB ÀÃdoes not lead to production of AB Àions because energy and momentum are not gen-erally conserved when two bodies collide elastically to form one body (see Problem3.12).Hence the excited AB ÀÃion separates,AB ÀÃÀ!e þABunless vibrational radiation or collision with a third body carries off the excess energy.These processes are both slow in low-pressure discharges (see Section 9.2).At high pressures (say,atmospheric),three-body attachment to form AB Àcan be very important.For a few molecules,such as some halogens,the electron afﬁnity of the atom exceeds the dissociation energy of the neutral molecule,leading to the potential energy curves shown in Figure 8.7c .In this case the range of electron impact ener-gies E for excitation of the AB Àrepulsive state includes E ¼0.Consequently,there is no threshold energy,and very slow electrons can produce dissociative attachment,resulting in hot neutral and negative ion fragments.The range of R s over which auto-detachment can occur is small;hence the maximum cross sections for dissociative attachment can be as high as 10À16cm 2.A simple classical estimate of electron capture can be made using the differential scattering cross section for energy loss (3.4.20),in a manner similar to that done for dissociation.For electron capture to an energy level E 1that is unstable to autode-tachment,and with the additional constraint for capture that the incident electron energy lie within E 1and E 2¼E 1þD E ,where D E is a small energy difference characteristic of the dissociative attachment timescale,we obtain,in place of (8.3.2),s att¼0E ,E 1s 0E ÀE 1E 1E 1,E ,E 20E .E 28>><>>:(8:3:5)8.3ELECTRON COLLISIONS WITH MOLECULES 249wheres 0%p m M R 1=2e 4pe 0E 1 2(8:3:6)The factor of (m =M R )1=2roughly gives the fraction of excited states that do not auto-detach.We see that the dissociative attachment cross section rises linearly at E 1to a maximum value s 0D E =E 1and then falls abruptly to zero.As for dissociation,E 1can depend strongly on the nuclear separation R ,and (8.3.5)must be averaged over the range of E 1s corresponding to the ground state vibrational motion;e.g.,from E thr to E 0thr in Figure 8.7a .Because generally D E (E 0thr ÀE thr ,we can write (8.3.5)in the forms att %p m M R 1=2e 4pe 0 2(D E )22E 1d (E ÀE 1)(8:3:7)where d is the Dirac delta ing (8.3.7),the average over the vibrational motion can be performed,leading to a cross section that is strongly peaked lying between E thr and E 0thr .We leave the details of the calculation to a problem.Polar DissociationThe process,e þAB À!A þþB Àþeproduces negative ions without electron capture.As shown in Figure 8.7d ,the process proceeds by excitation of a polar state A þand B Àof AB Ãthat has a separ-ated atom limit of A þand B À.Hence at large R ,this state lies above the A þB ground state by the difference between the ionization potential of A and the electron afﬁnity of B.The polar state is weakly bound at large R by the Coulomb attraction force,but is repulsive at small R .The maximum cross section and the dependence of the cross section on electron impact energy are similar to that of pure dissociation.The threshold energy E thr for polar dissociation is generally large.The measured cross section for negative ion production by electron impact in O 2is shown in Figure 8.8.The sharp peak at 6.5V is due to dissociative attachment.The variation of the cross section with energy is typical of a resonant capture process.The maximum cross section of 10À18cm 2is quite low because autode-tachment from the repulsive O À2state is strong,inhibiting dissociative attachment.The second gradual maximum near 35V is due to polar dissociation;the variation of the cross section with energy is typical of a nonresonant process.250MOLECULAR COLLISIONS。

Two photon amplitude of partially coherent partially entangled electromagnetic fields

Two photon amplitude of partially coherent partially entangled electromagnetic ﬁeldsMiguel Angel Olvera,Sonja Franke-ArnoldJuly 31,2015AbstractThe development of eﬃcient protocols for pure and mixed states prepa-ration is challenging task.Most of the theory of quantum information applications has been developed for fully coherent or completely incoher-ent light.However,in many situations of interest partially coherent light has been proved to be a more robust model of radiation.In this paper the underpinning theory of two photon amplitude functions for down-converted ﬁelds with partially coherent pump beams is investigated.By using the generalised Siegert relations and the coherent mode representa-tion of the cross spectral density matrix the two photon amplitude is fully characterised for partially coherent beams.A number of correlation prop-erties from modern coherence theory are demonstrated to be preserved under parametric down-conversion.Based on the generalised Siegert re-lations and the Cauchy-Schwarz inequality a measure of entanglement for the two photon amplitude is proposed.Upper bounds for this measure are found in terms of the golden ratio for maximally entangled states.Two inequalities are derived for the two photon amplitude for which there exist a transition zone from super-Poisson statistics to sub-Poisson statistics for down-converted partially coherent ﬁelds.1IntroductionThe two photon amplitude (TPA)plays a role of paramount importance in the concept of quantum entanglement which has been largely recognized as a fundamental property of the non linear phenomena known as parametric down conversion (PDC)[1].The widespread use of bi-photon generation techniques and the improvement of coincidence detection of photons has led to the un-derstanding of the entanglement of bipartite quantum systems as well as to the implementation of eﬃcient quantum information protocols.However in the vast majority of investigations,the pump ﬁeld is assumed to be coherent and the strategy consists in tailoring the TPA to investigate changes in the spectral properties of bipartite systems that can generate high dimensional entanglement or lead to new entanglement schemes [2,3,4,5,6].The aim of the present paper is to include the correlation properties of the pump ﬁeld in order to investigate correlation-induced entanglement eﬀects.TPA shape manipulation has been investigated by two main approaches,by changing the pump spectrum and by adjusting the phase matching conditions.The angular spectrum of the pump laser,preserved it the two photon state,1a r X i v :1507.08623v 1 [q u a n t -p h ] 30 J u l 2015allows taking control of the transverse correlations by transferring the image from the pump to the down-convertedﬁeld citePhysRevA.57.3123.Focusing a laser pumpﬁeld has led to the evaluation of the correlation function in an imaging system with two photon geometric optics eﬀects by passing the laser beam through a focusing lens before the down-conversion crystal[7].Down-converted photons have been used to investigate the eﬀect of pump spectrum and the various parameters of entangled-photon imaging systems on the imaging resolution[8].Entanglement enhancement has been investigated on the basis of a judicious selection of eigenmodes in angular momentum entanglement[6]. The characterization of entanglement by determining an appropriate set of bi-orthogonal mode pairs has been performed by using the Schmidt decomposition of the TPA[9].This approach has been used for engineering quantum correla-tions by controlling spectral amplitudes[10].The eigenvalues and eigenmodes of the density matrix have been used to approximate the TPA by a bi-Gaussian function and for calculating the Schmidt number[11].The two photon state can be controlled by using transversely patterned quasi-phase matching gratings to tailor the spatial mode function that describes the state of photon pairs in PDC[12].It has been shown that type-II down-converted photons with broadband pump exhibit a continuous superposition of two-photon states in which the probability amplitude for each state depends on the pump bandwidth whose envelope function determines the range of pump energies and the phase-matching function determines the energy spectrum[13].In all the approaches mentioned above,the common characteristic is the use of coherent sources from down-convertedﬁelds are obtained.An important number of potential sources,namely,partially coherent sources have not been included and only a few studies have put forward the connection between en-tanglement and partial coherence.The mathematical theory of duality between partial coherence and entanglement comprises the description of propagation, diﬀraction and interference of bi-photonﬁelds in optical linear systems[14]. Coherence properties of down-convertedﬁelds were investigated in terms of the physical properties of a coherent pump beam[15].In this connection,recently it was proved that down-converted photons retain the coherence properties of the pumpﬁeld and the maximum entanglement is bounded by the degree of spatial coherence[16].With the development of modern coherence theory,in the last decade a number of results and techniques have been established that brings deep insight into the concepts of coherence and polarisation expressed through the cross spectral density matrix[17,18,19,20,21,22,22,23,24,25].In the present paper theoretical implications of correlation-induced entan-glement eﬀects,i.e.,tailoring the TPA photon amplitude with partially coherent ﬁelds on bipartite partially entangled systems,are investigated.The paper is organized as follows.In section2it is demonstrated that the coherence proper-ties of partially coherent pumpﬁelds are preserved in the two photon entangled ﬁelds.In section3the cross spectral density operator is introduced.The ex-pected value of this operator is related to the two photon amplitude trough the kernel of the one photon amplitude.In section4an extension to the measure of entangled states proposed in[26]is established for the TPA of partially coherent ﬁelds that satisﬁes the Siegert relation.Under this formalism it is found that maximally entangled states have an upper bound given by the golden ratio.An important consequence is that entanglement beyond the golden ratio implies the violation of the Cauchy-Schwarz inequalities for maximally entangled states and2a transition from super-Poisson statistics to sub-Poisson is involved.Finally,in section5conclusions are presented.2Coupling of partially coherent down-converted ﬁeldsThe TPA contains all the information of the down convertedﬁeldsﬁeld at the moment of birth inside the non-linear material.The correlation time of the pumpﬁeld was believed to play no role in the two photon second order corre-lations,fundamentally because in Mandel’s original derivation the bandwidth was taken to be zero and in part because the concepts of partial coherence in the space frequency domain was not fully developed.Note that Wolf’s theory of partial coherence in the space frequency domain was published a year later than Mandel’s theory,in1986[1,27].In the vast majority of investigations concerned with two photon systems,the pump beam is assumed to be coherent. If the pump is partially coherent the two-photonﬁeld operator depends on the interaction time,the representation of the classicalﬁeld and the non linear prop-erties of the crystal.The expected value of theﬁeld operator depends on both the expected value of the quantumﬁeld operator and the cross spectral den-sity matrix.Partially coherentﬁelds pertain to a large class of electromagnetic sources that obey super-Poisson statistics although not fully unbiased,they are often referred to thermalﬁelds.Among the large class of partially coherent sources,here we are interested in partially coherentﬁelds for which the fourth order correlation functionΓ(2)(r1,r2) can be expressed through the second order correlation function,i.e.those for which the Siegert relation holds[28]Γ(2)(r1,r2)=Γ(1)(r1,r1)Γ(1)(r2,r2)+|Γ(1)(r1,r1)|2.(1) With this relations the fourth order correlation functions are characterised in terms of second order correlation functions.In the following the down-conversion of photons from the partially coherent pumpﬁeld will be analysed following Mandel’s original derivation[1]within the time where the interaction takes place.The analysis is valid for non-degenerate type I and type II SPDC.Consider an electromagneticﬁeld V l(r,t)entering a dielectric non linear crystal with bilinear susceptibilityχ,the Hamiltonian of the system isH=12DχijlˆU i(r,t)ˆU j(r,t)V l(r0,t)d r,(2)where D is the spatial domain where the interaction of light and the crystal takes place and V l(r0,t)=V l e[i(k0·r0−ω(k0)t)]is a member of the classical pump ﬁeld ensemble of realizations with average frequencyω0.The quantizedﬁeld is given byˆU(r,t)=1L3/2Dεk sˆa†k(k,t)e i(k·r)d k,(3) 3whereˆa†(k,t)is theﬁeld creation operator of the mode k,εk s is a unit polari-sation vector depending on the wavevector k,s=1,2is the polarization index and r spans the transverse plane perpendicular to the propagation axis.The Hamiltonian is then given byˆH=Dχijlˆa†k sˆa†k sV l(r0,t)ε∗ksiε∗k sje i[(k0−k−k )r−ω(k0)t]d r d k d k .(4)By deﬁningˆA=1/L3/2ˆa e iω(k)t the Heisenberg equations of motion can be written as follows˙ˆA=1i L3Dχijl k s,k s ˆA†k sV l(r0,t)(5)e i[(k0−k−k )·r]e−i[(ω(k0)−ω(k)−ω(k ))t]d r d k d k ,where k si,k s j represents the unit polarisation vector.In order to determine theﬁeld mode the Heisenberg equations of motion must be integrated over the interaction time∆t.If the pumpﬁeld is considered coherent with a coherence time of the order of microseconds and since the interaction time is typically a fraction of a nanosecond the time integral is straightforward and yields the familiar sinc function,whose argument contains the phase matching conditions, of the frequency and the interaction time.In the case of partially coherent sources the situation is diﬀerent.The values of temporal coherence ranges from a few to hundreds of femtoseconds for partially coherent sources and fractions of nanoseconds for multimode lasers.With the development of the modern co-herence theory[29],an important number of sources with prescribed properties of coherence has been put forward so that the range of temporal coherences available under the time integration is non negligible.Hence the integration over time is expressed as followsˆA=1i L3δtDχijlˆA†k sT ij(k s,k s )V l(r0,t )(6)e i[(k0−k−k )·r]e−i[(ω(k0)−ω(k)−ω(k ))t )]d r d k d k d t . It is convenient to express this integral as followsˆA=1i L3δtV l(r,t )e−i[ω(k0)−ω(k)−ω(k )]t d tDˆA†k se−i[k0−k−k ]r d rχijl T ij(k s,k s )d k d k ,(7) whereδt corresponds the interaction time.If the pumpﬁeld is partially coherent the contributions to the response operator of down-converted light at point r and time t resulting from the creation operator will be aﬀected by the correlations of the input beam within the non-linear material.To show how this happen, consider the operator4U(r,t)=U free(r,t)+ˆAk s˜V(r,ω)F(k,s,r,t)d k,(8)where U free is theﬁeld operator in the absence of any interaction,˜V(r,ω)is the Fourier spectrum of the inputﬁeld within the interaction intervalδt˜V(r,ω)=t0+δtt0V l(r,t)e−i[ω(k0)−ω(k)−ω(k )]t d t,(9),ˆA k s =ˆA†k se−i[k0−k−k ]r d r,(10)and F(k,s,r,t)is given byF(k,s,r,t)=1i L3χijl T ij(k s,k s )d k d k .(11)It was pointed out in the previous section that,by virtue of the Siegert re-lationΓ(2)(r1,r2)=Γ(1)(r1,r1)Γ(1)(r2,r2)+|Γ(1)(r1,r1)|2,the fourth order correlation function of partially coherent beams is fully described by the second order correlation function.From the present analysis is clear that the averaged measure of light intensity,at points(r1,t)and(r2,t)for t>δt,is given by Γ(2)(r1,r2)= U†(r1,t)U†(r2,t)U(r2,t)U(r1,t) ,therefore it is fully charac-terised by the second order correlation function given byΓ(1)(r1,r2;ω)=kkˆA†k sˆAk s˜V∗(r1,ω)˜V(r2,ω)F∗(k,s,k ,s ,r1,t)F(k,s,k ,s ,r2,t)d k d k .(12) Theﬁrst term in the integrand governs the quantum system of down converted photons,it depends on the vacuum expectation value of the boson operators during the interaction time and the phase matching conditions.The second term,recognised as the cross spectral density function of an electromagnetic ﬁeld,is the correlation function that governs the spatial coherence properties of the classical pumpﬁeld at frequencyω.Therefore the expected value of the two photon amplitude contains the information about both the down converted photons and the classical correlation properties of the pumpﬁeld.This result reveals the dual world to which down-converted photons belongs,when the pumpﬁeld is partially coherent the contribution to the two photon amplitude is much richer than the previous case.We shall see in the next section that in order to quantify the eﬀect ofﬁeld correlations in down-converted photons the cross spectral density matrix can be conveniently expressed as a density operator allowing the evaluation of the second order correlation function.3The cross spectral density operatorIt is well known that the second order correlation function satisﬁes the Wolf equations and the Helmholtz equation.The Wolf equations have a quantum5counterpart whose solution are the TPA;as a consequence the fourth order cor-relation function also satisﬁes the Helmholtz equation[28].This remarkable result constitutes a further demonstration of the duality between two photon systems and partially coherentﬁelds.These equations posses identical math-ematical structure in both position and Fourier space,besides both share the hallmark of quantum-optical systems namely,linearity.The solutions are there-fore connected and describe mathematically equivalent physical phenomena. The second order coherence function represents the oscillatory behaviour of partially coherent light in propagation and diﬀraction.The two photon am-plitude represents the probability amplitude of oscillation modes in the system of down-converted photons.Therefore the two photon Wolf equations imply that the TPA admits a modal representation in terms of the eigenvalues and eigenvectors of the second order correlation function.Despite of this compatibility further clariﬁcation is necessary.The second order correlation function and the cross spectral density function are correlation functions with analogous mathematical deﬁnition,however do not describe the same optical phenomena.The Young interference experiment reveals theﬁeld source correlations at the plane of interference.The mathematical description of the interference pattern involves two types of coordinates,i.e.,the intensity,in terms of coordinates from the plane of interference,is weighted by the degree of coherence in terms of coordinates from the source plane.This subtle distinction reveals the nature of the coherence functions as source correlation functions.The degree of coherence does not provide any information of correlations that may occur at the interference plane,where the light is detected.The second order correlation function describes correlations of arrivals,i.e.,detection correlations and the same holds for the fourth order correlation function.In this regard do not contain information about correlations at the source plane because they are in terms of photon counting statistics.For the sake of comparison it is possible to calculate the degree of coherence at the interference plane,the result is that in general is diﬀerent from the source.The same holds in the other case,intensity correlations can be measured at the source plane but in general these will not be equal to the measurements at the detection plane.In the following we proceed to elucidate the connection between the second order correlation function and the cross spectral density matrix in the context of spontaneous parametric down conversion for partially coherent light.One of the most important results in vector coherence theory states that when the cross-spectral density(CSD)matrix satisﬁes square integrability:D TrW†(r1,r2;ω)W(r1,r2;ω)d r1d r2<∞,(13)hermitian symmetry:W†(r1,r2;ω)=W(r2,r1;ω),(14) and is non-negative deﬁnite:Df(x1)W(r1,r2;ω)f(x2)d r1d r2≥0,(15)6where f(r)is an arbitrary row matrix,hence the CSD becomes a Hilbert-Schmidt operator.This is an important result because the CSD functions can be ex-panded in Mercer’s series[30,31]W(r1,r2;ω)=nΛnΦ†n(r1)⊗Φn(r2),(16)where coeﬃcientsΛn are the eigenvalues andΦn(r)=[ϕn,i(r)]are the eigen-vectors of the Fredholm integral equation given byDΦn(r1)W(r1,r2;ω)d r1d r2=ΛnΦn(r2).(17)Because of relations(13),(14)and(15)the eigenvaluesΛn are real and non-negative,and the eigenvectorsΦn(r)are orthonormal.Therefore,decomposi-tion(16)represents the cross spectral density matrix of any partially coherent and partially polarised electromagneticﬁeld as a superposition of completely coherent completely polarised modes.The vector integral equation(17)repre-sents a set of two coupled scalar integral equations whose kernel W(r1,r2;ω)is for the general case unknown.However considerable simpliﬁcation is obtained when the kernel is diagonalised,in such a case the integral equations uncouple and can be treated independently.Solving the integral equation(17),for any particular case is not an easy task.For those cases in which this can be achieved,a fundamental property of Mercer’s series is that they are absolutely and uniformly convergent.The eigenvalue distribution typically can involve a large number of elements,this fact makes possible to express the m th-eigenvalue for the i th-component of matrix (16)in terms of power series.By the central limit theorem,the expansion of the m th-eigenvalue is of the formΛm,i(λ)=ml=0(−1)lλ2l il!.(18)The parameterλi,that satisﬁes0≤λi≤1,depends on the ratio between the eﬀective width of the sourceσs and the correlation lengthσc in a functional form determined by the particular type of source.The power series expansion represents the contribution,per number of photons,of the m th-mode to the coherence of theﬁeld.The second order correlation function expressed in terms of the density operatorˆρis given by˜V∗i(r1;ω)˜V j(r2;ω) =qφq|ˆρ˜V∗i(r1;ω)˜V j(r2;ω)|φq ,(19) whereˆρ=∞n=0P n(α)|α α|.(20) 7Therefore the modal expansion determines uniquely the contribution of modes per number of photons to the coherence of the ﬁeld.The eigenvalue expansion in the coherent mode representation is related to the coherent state representation as followsq φq |ρ˜V ∗i (r 1;ω)˜V j (r 2;ω)|φq =∞ m =0m k =0(−1)k λ2k i k !φm,i (r 1)φ∗m,i (r 2).(21)By using the photon number operator,which satisﬁes a †a |m =m |m ,it follows from Eq.(21)that the cross spectral density operator,say ˆµii ,is given byˆµii =e −[ˆa †k i ˆa k i In(λi )]2.(22)Therefore,the expected value of the cross spectral density function is found to beTr {ˆρˆµii }=e −(α2k +α4k )In(λi )2.(23)The density operator ˆρis represented as a superposition of coherent states which form an overcomplete basis but they are non orthogonal,the lack of orthogo-nality of the coherent state representation also holds and is revealed by the fact that the basis of the eigenvalues power expansion is also non-orthogonal.From Eq.(23)it can be seen that if λ→1the ﬁeld is completely coherent and consists of only one mode for a given number of photons,the corresponding CSD can therefore be factorized.If λ→0the ﬁeld consists of a superposition of inﬁnite modes weighted by their corresponding eigenvalues and turns out to be incoher-ent.The larger the number of modes,the smaller number of photons per mode so that the corresponding CSD can no longer be factorized.In the more general and realistic case,the pump ﬁeld is partially coherent,the expected value is then formed by a superposition of several modes and depending on the strength of the eigenvalues,the number of photons per mode contribute with diﬀerent amounts to the ﬁeld coherence.It is important to note that down-conversion eﬃciency relies on the phase matching conditions,when these are fulﬁlled and plane waves are used as pump,the sinc function attains its maximum value.If the pump ﬁeld is partially coherent this situation changes,Eq.(23)shows that the expected value of the cross spectral density operator attains its maximum value for a larger frequency range centred at the phase matching condition.As a consequence maximum eﬃciency can be attained for partially coherent beams for a given mean frequency of the pump beam.In ﬁgure 1we compare the contribution of the typical sinc function versus the expected values of the CSD density operator.It can be seen that for completely coherent ﬁelds the sinc function imposes a natural limit for which the states be-come rather constrained.If partially coherent light is employed,this restriction no longer appears and a number of new states are feasible.In particular for incoherent ﬁelds,i.e.,when λi →0,the two photon amplitude approaches to a delta function,the coherence properties can therefore be engineered a priori opening new possibilities to attain maximally entangled states.In this regard8Figure1:Expected values of the cross spectral density operator for diﬀerent values ofλ.Cohrent caseλi→1is represented by large-dashed curve.Partially coherent caseλi=0.5is represented by medium-dashed curve.Incoherent case λi→0is represented by short-dashed curve.For comparison,solid line is the typical sinc function for the coherent case.Inﬁgureκ=k0−k−k .a relationship between entanglement,the two photon amplitude and partially coherentﬁelds will be investigated in the next section.Now some consequences of the inclusion of the correlation properties will be shown.On substituting Eq.(23)into(24)the second order correlation function isΓ(1)(r1,r2;ω)=δkk Tr{ˆρˆµii}F∗(k,s,k ,s ,r1t)F(k,s,k ,s ,r2,t)d k d k .(24)The two photon amplitude now exhibits a more involved phenomenon.For t>∆t it describes the spectral contribution of coherence to the probability of detecting one photon at r with frequencyω.A CSD is called genuine if it satisﬁes the non negative deﬁniteness condition[32],this is a fundamental feature of all physically realizable correlation func-tions.However not every correlation function associated with an electromag-neticﬁeld satisﬁes this condition.A necessary and suﬃcient condition states that a correlation function represents a genuine CSD if and only if it can be formally expressed in the form[19,20]W(r1,r2;ω)=p(v1,v2)H∗(r1,v1;ω)H(r2,v2;ω)d v1d v2.(25)This important result in modern coherence theory states that the CSD pertains to a class of functions in a reproducing kernel Hilbert space(RKHS)and satisﬁes the reproducing property(17).Since both correlation functions in the integrand of Eq.(24)satisfy the non negativity requirement,and because A†kA k =δkk ,9their product can be regarded as the product of two reproducing kernels in the more general Hilbert space H =H Q ⊗H S .Functions pertaining to the space H Q are functions in the quantum ensemble of states and functions pertaining to the space H S are functions of the statistical ensemble of realizations.By the integral representation theorem for reproducing kernels [33],the product of the correlation functions in the integrand of Eq.(24)is a reproducing kernel.Therefore,on substituting Eq.(22)into Eq.(24)the functionΓ(1)(r 1,r 2;ω)= e −(α2k +α4k )In(λi )2F ∗(k ,s,r 1,t )F (k ,s,r 2,t )d k (26)do satisfy the property (25)for v 1=v 2=k and indeed becomes a reproduc-ing kernel.Hence the dual property of the quantum nature of down-conversion process and classical correlations are contained in the new reproducing ker-nel Hilbert space of the two photon amplitude.The two photon amplitude Γ(2)(r 1,r 2;ω)contains all the information of the classical and quantum system through the one photon amplitude Γ(1)(r 1,r 2;ω)which describes the joint con-tribution of the classical correlation functions to the two photon amplitude of down converted photons.It could be tempting a decomposition of the TPA similar to Eq.(16),how-ever,since the TPA in general is not hermitian,a Schmidt decomposition [34]is possible instead.In the next section it will be shown that such a a decom-position implies a link between partially coherent ﬁelds and non-classical ﬁelds,i.e ﬁelds with super Poisson statistics and ﬁelds with sub-Poisson statistics.4Partially correlated ﬁelds and quantum entan-glementThe results of the previous sections can be exploited to investigate the eﬀect of correlation properties of the pump beam on the two photon wave function.In coherence theory two relationships between the eigenvalues and the properties of the CSD matrix,namelyTr[W †(r 1,r 2;ω)W †(r 1,r 2;ω)]d r 1d r 2=n Λ2n ,(27)andTr[W †(r ,r ;ω)]d r =n Λn ,(28)lead to the deﬁnition of the eﬀective degree of coherence [35,36]µeff = n Λ2n n Λn 2.(29)This value represents a relative measure of coherence within a speciﬁc area.Note that the term 1/ Λn corresponds to the Schmidt number which is a10measure of entanglement.The eﬀective degree of coherence and the Schmidt number seem to play equivalent role for coherence and entanglement.In the present case,the eﬀective degree of coherence(29)can be calculated from the eigenvalue power expansion(18),substituting Eq.(18)into(29)the eﬀective degree of coherence is given byµeff= ∞n=0nm=0(−1)mλ2m2m!2∞n=0nm=0(−1)mλ2m2m!2.(30)In general the eigenvalues depend on the ratioλ=σwσcand are determinedfundamentally by the analytic form of the CSD matrix.It is clear that for a given genuine CSD this result shows the fact that there exist an eﬀective number of modes with a deﬁned number of photons per mode as stated by Eq.(23).It may be tempting to represent the TPA in the form(16),however the lack of hermiticity imposes a rather strong condition for the implementation of a modal expansion therefore the two photon system does not admit a coherent mode representation but a Schmidt decomposition.The main distinction between these decompositions relies in the fact that the two photon system Hilbert space comprises the tensor product of Hilbert spaces of functions u,v∈H Q and x,y∈H S.A pure bipartite system is given byξ=αx⊗u+βy⊗v.(31) In the Schmidt basis the density matrix of the system is given by[34]ρ=ξξ†=i,j c i c∗jαu i u†j⊗v i v†j,(32)which can be expressed as the product of reduced density matrices of the form (16).This implies that whereas non hermiticity of operators and non orthogo-nality of bipartite system basis do not allow us to express Eq.(1)in the form (16),the reduced density matrix admits a Mercer’s series provided hermiticity, orthogonality and square integrability is fulﬁlled in each subsystem.An expan-sion of the form(32)for entangled states of bipartite systems has been proposed to express the system as the sum of a completely entangled states and a factor-izable state[26].In the same spirit and by taking into account that for partially coherentﬁelds the Siegert relations holds,we propose a Schmidt decomposition for the TPA as the sum of a factorizable non entangled amplitude function plus a completely entangled amplitude function of the formΓ(2) E (r1,r2)=M EΓ1e(r1,r2)+1−M2EΓ1f(r1,r2),(33)with the entangled componentΓ1e(r1,r2)=|Γ1(r1,r2)|2,(34)11。

半导体光谱共聚焦

半导体光谱共聚焦英文回答：Semiconductor spectroscopy is a powerful technique that allows us to study the interaction between light and semiconductors. It provides valuable information about the electronic and optical properties of semiconductors, which is crucial for the development of various electronic devices.One of the advanced techniques used in semiconductor spectroscopy is confocal spectroscopy. Confocal spectroscopy combines the principles of confocal microscopy and spectroscopy to achieve high spatial and spectral resolution. It allows us to collect emission or absorption spectra from a specific region of interest in a semiconductor sample.In confocal spectroscopy, a laser beam is focused onto the semiconductor sample using a high numerical apertureobjective lens. The emitted or transmitted light from the sample is collected through the same objective lens and directed towards a spectrometer for analysis. By using a pinhole aperture in front of the detector, out-of-focuslight is rejected, resulting in improved spatial resolution.Confocal spectroscopy offers several advantages over conventional spectroscopy techniques. Firstly, it provides high spatial resolution, allowing us to study localized regions within a semiconductor sample. This is particularly useful when investigating the electronic and optical properties of nanostructures or devices with submicron dimensions.Secondly, confocal spectroscopy allows us to obtain spectra from specific depths within a semiconductor sample. By adjusting the focus of the objective lens, we can selectively collect spectra from different layers or interfaces. This is important for understanding the optical properties of layered structures, such as quantum wells or heterojunctions.Furthermore, confocal spectroscopy can be combined with other microscopy techniques, such as scanning probe microscopy or fluorescence microscopy, to provide complementary information. For example, by combining confocal spectroscopy with scanning tunneling microscopy,we can simultaneously measure the electronic and optical properties of a semiconductor surface with high spatial resolution.In summary, semiconductor spectroscopy and confocal spectroscopy in particular, are powerful tools for studying the electronic and optical properties of semiconductors. They provide valuable insights into the behavior of semiconductors at the nanoscale and are essential for the development of advanced electronic devices.中文回答：半导体光谱共聚焦是一种强大的技术，可以研究光与半导体之间的相互作用。

双光子显微镜在小动物活体光学成像中的研究进展

双光子显微镜在小动物活体光学成像中的研究进展张文豪;李建军;杨德刚;杨明亮;杜良杰;高峰;刘长彬;李大鹏;胡安明【摘要】双光子显微镜结合了激光扫描共聚焦显微镜和双光子激发技术.双光子荧光显微镜具有光损伤小、漂白区域小、穿透能力强、高分辨率、荧光收集率高、图像对比度高、可实现暗场成像、适合多标记复合测量等多种特点,可应用于小动物活体光学成像,在肿瘤免疫治疗、基因治疗、干细胞研究、药物筛选与评价、活体脊髓损伤成像等领域研究中有广泛应用.%Two-photon microscopy is a new technique which combines laser scanning con-focal microscopy and two-photon excitation technique. Two-photon fluorescence microscopy has the advantages of little light damage, small bleaching area, strong penetrability, high resolution, high fluorescence collection efficiency, and high image contrast. It is suitable for dark field imaging and multi-labeled compound measurement, and has been widely used in small animals in vivo optical imaging, such as research for tumour, gene therapy, stem cells, drug development, spinal cord injury, etc.【期刊名称】《中国康复理论与实践》【年(卷),期】2017(023)001【总页数】5页(P37-41)【关键词】双光子显微镜;活体光学成像;小动物;综述【作者】张文豪;李建军;杨德刚;杨明亮;杜良杰;高峰;刘长彬;李大鹏;胡安明【作者单位】首都医科大学康复医学院,北京市 100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市 100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市 100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市 100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市 100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市 100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市 100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市 100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市100068;中国康复研究中心北京博爱医院,脊柱脊髓神经功能重建科,北京市100068;北京脑重大疾病研究院神经损伤与修复研究所,北京市 100068;北京市神经损伤与康复重点实验室,北京市 100068;首都医科大学康复医学院,北京市100068;中国康复研究中心北京博爱医院,神经外科,北京市 100068【正文语种】中文【中图分类】R446.8[本文著录格式] 张文豪，李建军，杨德刚，等.双光子显微镜在小动物活体光学成像中的研究进展[J].中国康复理论与实践, 2017,23(1):37-41.CITED AS:Zhang WH,Li JJ,Yang DG,et al.Research progress of two-photon microscopy in small animals in vivo imaging(review)[J].Zhongguo Kangfu Lilun Yu Shijian,2017,23(1):37-41.1931年，Maria Göppert-Mayer在博士论文中第一次提出原子或分子双光子激发的理论假设。

多光子态的干涉和区分

附件2论文中英文摘要作者姓名：孙方稳论文题目：多光子态的干涉和区分作者简介：孙方稳，男，1979年10月出生，2004年9月师从于中国科学技术大学郭光灿教授，于2007年6月获博士学位。

中文摘要光学，作为一种工具，在物理学的基础研究和各种应用领域中都发挥着巨大的作用。

特别是在激光被发明后，很多重要的研究和发现都是以它为基础完成的。

而光的相干性研究又是光学应用的基础，对于研究物理学基础领域例如各种物质与光的相互作用有重要的用处；在应用领域，光的相干性研究对于高精密测量、图象处理等都有重要的推动作用。

此外，在新兴的量子信息技术中，光的相干性研究也起到了重要的推动作用。

在量子信息技术中, 量子力学的量子态叠加理论和测量塌缩理论保证了量子密钥分配的安全；同样量子态叠加理论和演化理论使得量子计算可以并行工作，其效率将远优于普通电子计算机。

在实验上，人们尝试着在多种物理体系上实现量子通讯和量子计算。

而光学方法因为其独特的优点，较其他方法发展更快。

首先，光子的易传输性是其他物理体系不具备的。

因此在实际量子密钥分配中，几乎都使用光子作为信息载体。

其次，成熟的非线性光学手段和良好的激光光源使得光子态制备变的容易。

最后，对于光子态的精密控制和探测也使得人们容易完成不同的量子操控方案。

所以，诸如量子密钥分配，量子力学非局域性检验，量子隐形传态等一系列实验都是首先利用光子完成的。

实际上，用在量子信息中的光子相干特性在物理本质上就是某种光子干涉，都是建立在各种干涉模型上的。

如实际的量子密钥分配的光学部分就是一种单光子干涉；而利用线性光学方法来完成的量子计算方案是多光子干涉。

因此对光子干涉本身的研究将为其在量子信息中的应用提供更好的方法。

反过来，光子干涉也是一种量子操作过程。

我们可以利用量子信息的语言来描述和分析干涉。

例如光子干涉时对态的转换可以表示成量子信息中的量子比特操作；而其干涉可见度的不同就是量子信息中的消相干作用的结果。

Anti-Stocks位移的原理与应用

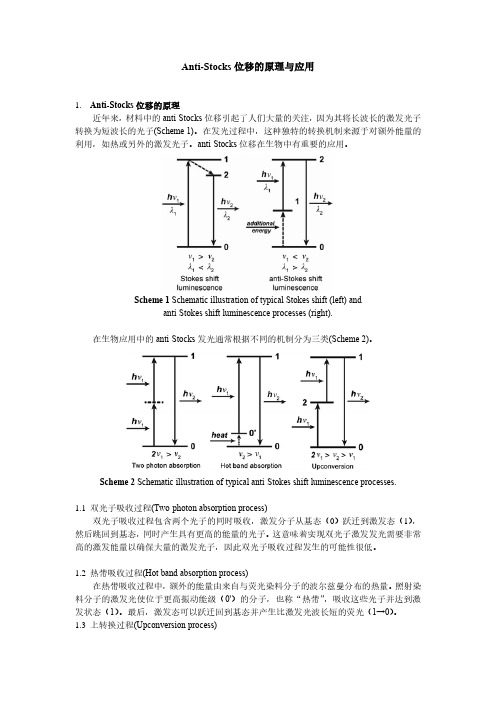
Anti-Stocks位移的原理与应用1.Anti-Stocks位移的原理近年来，材料中的anti-Stocks位移引起了人们大量的关注，因为其将长波长的激发光子转换为短波长的光子(Scheme 1)。

在发光过程中，这种独特的转换机制来源于对额外能量的利用，如热或另外的激发光子。

anti-Stocks位移在生物中有重要的应用。

Scheme 1 Schematic illustration of typical Stokes shift (left) andanti-Stokes shift luminescence processes (right).在生物应用中的anti-Stocks发光通常根据不同的机制分为三类(Scheme 2)。

Scheme 2 Schematic illustration of typical anti-Stokes shift luminescence processes.1.1双光子吸收过程(Two-photon absorption process)双光子吸收过程包含两个光子的同时吸收，激发分子从基态（0）跃迁到激发态（1），然后跳回到基态，同时产生具有更高的能量的光子。

这意味着实现双光子激发发光需要非常高的激发能量以确保大量的激发光子，因此双光子吸收过程发生的可能性很低。

1.2热带吸收过程(Hot band absorption process)在热带吸收过程中，额外的能量由来自与荧光染料分子的波尔兹曼分布的热量。

照射染料分子的激发光使位于更高振动能级（0'）的分子，也称“热带”，吸收这些光子并达到激发状态（1）。

最后，激发态可以跃迁回到基态并产生比激发光波长短的荧光（1→0）。

1.3上转换过程(Upconversion process)在上转换过程中，发光中心可以吸收两个或更多光子的能量（0→2→1），然后产生比每个单独的激发光子更高能量的发射光子（1→0）。

刺激响应性聚集诱导发光凝胶因子的设计、合成及性能

2023年6月裴强等：刺激响应性聚集诱导发光凝胶因子的设计、合成及性能的聚集诱导发光性质。

在紫外灯（365nm ）下观察，凝胶因子G1在能形成凝胶的溶剂（环己烷、DMSO ，1×10-5 mol/L ）中发出明亮的蓝光，而在不能形成凝胶的溶剂（CH 2Cl 2、CHCl 3，1×10-5mol/L ）中不发光[图4(c)]。

可能原因是，凝胶因子G1在环己烷和DMSO 中溶解性较差，形成了聚集体，从而显示出聚集诱导发光现象。

相反，G1在CH 2Cl 2、CHCl 3中溶解性好而无法形成聚集体，因此溶液没有荧光。

荧光光谱研究发现，凝胶因子G1在能够聚集形成凝胶的两种溶剂中荧光强度增大，且最大发射波长明显蓝移[图4(d)]。

荧光光谱研究结果与365nm 紫外线下观测的结果保持一致。

2.4 红外光谱和变温核磁红外光谱和核磁共振技术被广泛应用于超分子凝胶体系的研究之中，它可以提供关于分子组装方面的重要信息[21]。

从化合物G1在DMSO 中形成的干凝胶的红外光谱图可以看出，3286cm -1处有强吸收峰，可以归属为酰胺键中的N —H 键的伸缩振动峰。

此外，在1633cm -1处观察到较强的C =O 伸缩振动吸收峰。

与游离的酰胺键中的N —H （3400cm -1）和C =O （1680cm -1）伸缩振动峰相比，这两个峰明显向低场移动，且吸收强度增强，这可能是由酰图2　G1和G2的合成路线图表1　凝胶因子G 1和G 2在不同溶剂中的凝胶性能溶剂环己烷苯甲苯二甲苯乙腈乙酸乙酯丙酮四氢呋喃甲醇DMSO DMF二氯甲烷氯仿H 2OG1G(5.6)S S SP P S S PG (16.7)S S S IG2S S S S IP P S P P S S S I 注：G 为凝胶；S 为溶解；I 为不溶；P 为沉淀；括号里面的数值为临界成胶浓度（mg/mL ）。

··3109化工进展， 2023， 42（6）胺键中的N —H 与羰基O 分子间的氢键作用导致的[图5(a)][24-27]。

SEMICONDUCTOR LASER BY TWO PHOTONS TRANSITION

ቤተ መጻሕፍቲ ባይዱ专利内容由知识产权出版社提供
专利名称：SEMICONDUCTOR LASER BY TWO PHOTONS TRANSITION
发明人：YAMADA MINORU 申请号：JP16133879 申请日：19791212 公开号：JPS5683986A 公开日：19810708
摘要：PURPOSE:To make it possible to actuate a laser even in an indirect transition material by a method wherein two kinds of laser actions are performed at the same time between two pairs of levels with the energy level of an impurity or an independent atom as an intermediate level. CONSTITUTION:In case of the indirect transition material, if a level 4 of an impurity or independent atom is taken as an intermediation, electrons must thoroughly be supplemented for the level 4 to permit the laser actions to be performed in the transition from a conductor 1 to a valence electron zone 3, and a period of time required for the supplement must be longer than the transition time of 4 3. For this reason, the two kinds of the laser actions are performed at the same time between the two pairs of the levels of 1 4 and 4 3, by using a semiconductor material in which the levels of the impurity and independent atom exist. That is, if the laser actions are performed between the levels of 1 4, electrons are thoroughly supplied for the level 4 and further, the laser actions can be performed between the levels 4 3 also. Accordingly, even such an indirect transition material permits the laser actions to be performed.

水热法合成双发射碳点的研究

水热法合成双发射碳点的研究王晨星;魏建航;白状伟【摘要】制备了一种新型的具有双发射波长的荧光碳点(CDs).以柠檬酸和苯并咪唑为原料,以甲酰胺为溶剂,采用一锅煮水热法合成.通过紫外-可见吸收光谱和荧光光谱对其光学性能进行研究,透射电子显微镜、X-射线衍射、拉曼光谱和傅里叶变换红外光谱等方法对碳量子点组成和形貌进行表征.结果表明,制备的CDs呈现蓝色荧光和红色荧光,在水溶液中有良好的分散性和光稳定性.合成的CDs发射的红光比蓝光对酸碱有着更高的灵敏性.红光发射在碱性中荧光较强,在酸性介质中荧光减弱,展现了潜在的pH传感性能.合成方法简单,对实现大批量合成双发射碳点提供一种新的思路.【期刊名称】《延安大学学报（自然科学版）》【年(卷),期】2019(038)001【总页数】5页(P47-51)【关键词】碳点;双发射;荧光【作者】王晨星;魏建航;白状伟【作者单位】西安科技大学化学与化工学院,陕西西安710054;西安科技大学化学与化工学院,陕西西安710054;西安科技大学化学与化工学院,陕西西安710054【正文语种】中文【中图分类】O613.71;O622.6碳量子点(CDs)因优越的光学性质、良好的生物相容性和多官能团修饰特性[1-5]广泛应用在传感、生物成像、药物递送、太阳能电池、光电子和光催化等研究领域[6，7]。

传统的单发射CDs，一般发射单色蓝光，因为生物底物在紫外光激发会产生蓝色自荧光性质，同时也会对生物组织造成一定程度的光损伤，所以限制了其在生物成像过程中的应用。

除此之外，这些单发射CDs也容易受到环境影响和激发波长，相似组份和传感器浓度等因素的影响[8，9]。

在另一方面，发红光的CDs具有良好的器官穿透性，并且对生物组织光损伤是微乎其微的。

所以，开发新的双发射CDs可以有效避免单发射CDs的缺点，为生物或生物医学分析提供更好的传感策略。

本文采用一种简单、低成本的水热法，一步合成双发射的碳量子点，该方法制备的CDs，水溶性良好，在465 nm和588 nm均有发射峰，并且发射峰在588 nm 时，对pH有良好的响应能力。

尼康双光子显微镜使用说明书

Nikon 2 Photon manual1.Turn on the button on the power extension cord.2.Switch on PCs (windows 7 on both), password Vilkommen2014.3.Check beam splitter in front of the detector, choose the one you need.4.Switch on an Andor camera (left from the Nikon microscope)5.Turn on Mai Tai laser: wait for warm up and then turn the emission on (COM port 1, power>2.7 is good), choose your wavelength (λ).6.Start Micromanager, use config TP_March2018 for two photon measurements, SH,G chooseEYE and focus on your sample (eye). Filters to use UV, FITC(495/519), TRITC (550/573), and Cy3 (549/562).7.For two photon measurements, switch on PMT2 software as admin on the left PC. Use configFTF5ZV6Y. Adjust wavelength according to the laser set wavelength (λ), switch on PMTs and HV, and adjust laser intensity (0-10).8.Start VistaVision software; go to Imaging if you need or to FastFlim.9.For measurements Choose filters for Channel 1 (direct) and for Channel 2. Dichroic mirrorOUT on the stage for λ in 800 range. Open Mai Tai laser shutter, choose two-photon option in Micromanager, open a shutter in micromanager and make Laser ON in PMT2.10.For FLIM measurements: choose external clock for 80MHz, then upload and do calibrationwith you substance (Florocine 4.05ns lifetime, Rhodamine B 1.68ns lifetime etc). Aftercalibration do your FLIM measurements.11.To turn off: Close shutter, switch off the laser, close Mai Tai software, close micromanager,VistaVision, PMT2, switch off Andor camera and switch off power extension cord.12.Do the data transfer through VDS (use icon on the desktop-right click), fill the paper log.In the case of problems contact: Vita Solovyeva (************.dk) or Jonathan Brewer(**************.dk)。

由双暗态引起的窄谱线增强和双光子透明现象

由双暗态引起的窄谱线增强和双光子透明现象杨绍海;邓黎;周真【摘要】原子相干和量子干涉效应正逐步成为共振非线性光学物理的一种重要而且广泛的应用工具.在光学介质的非线性光学性质的研究中,在单光子、双光子和多光子吸收的禁止方面这些效应具有潜在的应用价值.尤其在光子比较少的光学领域,我们已经观察到了由电磁诱导透明的特大Kerr非线性特征和折射率增大而引起的无吸收非线性光学,这对量子非线性光学性质的研究具有极其重要的作用.【期刊名称】《华东交通大学学报》【年(卷),期】2010(027)003【总页数】5页(P88-91,101)【关键词】双暗态;电磁诱导透明;双光子透明【作者】杨绍海;邓黎;周真【作者单位】华东交通大学基础科学学院,江西南昌,330013;华东交通大学基础科学学院,江西南昌,330013;华东交通大学基础科学学院,江西南昌,330013【正文语种】中文【中图分类】O431.2近几年来，各种各样的被3个驱动场驱动的四能级原子系统已经表明可以用双暗态共振来刻画探测吸收。

由于双暗态共振的存在，基本上有两种原子结构:(1)Λ型结构系统，末态是双重能级;(2)三脚架原子系统。

双暗态的相互作用会引起一些重要的效应。

我们在这里研究了存在双暗态共振的五能级原子系统的双光子吸收光谱双光子吸收的抑制的两频率透明发生在这样一个系统中:一是拓展了双光子[1，2]透明的频率范围，二是改善了双光子透明的控制能力。

可以通过适当调节频率失谐量和两耦合场的强度来控制量频率透明窗的位置和宽度，并且在双光子吸收谱中可以看到一个强窄中心谱线，它可能在精细光谱学中得到应用。

最近，利用电磁诱导透明[3-5]，Wu等人研究并讨论了一个五能级原子系统的四波混频装置以及共振介质中的超拉曼散射，这引起了四波混频和超拉曼散射装置中的双光子吸收和三光子吸收被禁止，并使得四波混频在真实的共振中间态可以发生无吸收损耗。

1 理论模型和运动方程如图1所示，我们考虑的是一个由4个相干激光场驱动的五能级原子系统。