美国数学竞赛答案

合集下载

2019年美国数学竞赛(AMC10A)的试题与解答

(
)
0(19 ) 译文: 2
+ ((20)1)9 的值是多少?
解原式 = 20 + 19 = 2, 故 (C) 正确.
2. What is the hundreds digit of (20! − 15!)?
(A) 0 (B) 1 (C) 2 (D) 4 (E) 5
译文: (20! − 15!) 的百位数是多[ 少]?
years, n years apart. year Ana was 5 times as old as Bonita.
This year Ana’s age is the square of Bonita’s age. What is n?
(A) 3 (B) 5 (C) 9 (D) 12 (E) 15
equidistant from all four vertices of the quadrilateral?
— a square
— a rectangle that is not a square
— a rhombus that is not a square
— a parallelogram that is not a rectangle or a rhombus
13 个蓝球, 11 个白球和 9 个黑球. 在不放回的情况下, 必须
至少从盒子中取出多少个球才能确保会取出 15 个同种颜色
的球?
解依题意, 红球、绿球、黄球得取出 14 个, 蓝球、白球、
黑球全部取出, 再加任意 1 个就符合条件, 即至少需要取出
14 × 3 + 13 + 11 + 9 + 1 = 76 个球, 故 (B) 正确.

AMC 美国数学竞赛 2004 AMC 10B 试题及答案解析

2004 AMC 10BProblem 1Each row of the Misty Moon Amphitheater has 33 seats. Rows 12 through 22 are reserved for a youth club. How many seats are reserved for this club?SolutionThere are rows of seats, giving seats.Problem 2How many two-digit positive integers have at least one 7 as a digit?SolutionTen numbers () have as the tens digit. Nine numbers () have it as the ones digit. Number is in both sets. Thus the result is .Problem 3At each basketball practice last week, Jenny made twice as many free throws as she made at the previous practice. At her fifth practice she made 48 free throws. How many free throws did she make at the first practice?SolutionAt the fourth practice she made throws, at the third one it was , then we get throws for the second practice, and finally throws at the first one.Problem 4A standard six-sided die is rolled, and P is the product of the five numbers that are visible. What is the largest number that is certain to divide P?Solution 1The product of all six numbers is . The products of numbers that can be visible are , , ..., . The answer to this problem is their greatest common divisor -- which is , where is the least common multiple of . Clearly and the answer is .Solution 2Clearly, can not have a prime factor other than , and .We can not guarantee that the product will be divisible by , as the number can end on the bottom.We can guarantee that the product will be divisible by (one of and will always be visible), but not by .Finally, there are three even numbers, hence two of them are always visible and thus the product is divisible by . This is the most we can guarantee, as when the is on the bottom side, the two visible even numbers are and , and their product is not divisible by .Hence .SolutionProblem 5In the expression , the values of , , , and are , , , and , although not necessarily in that order. What is the maximum possible value of the result?SolutionIf or , the expression evaluates to .If , the expression evaluates to .Case remains.In that case, we want to maximize where .Trying out the six possibilities we get that the best one is, where .Problem 6Which of the following numbers is a perfect square?SolutionUsing the fact that , we can write:▪▪▪▪▪Clearly is a square, and as , , and are primes, none of the other four are squares.Problem 7On a trip from the United States to Canada, Isabella took U.S.dollars. At the border she exchanged them all, receiving Canadian dollars for every U.S. dollars. After spending Canadian dollars, she had Canadian dollars left. What is the sum of the digits of ?SolutionSolution 1Isabella had Canadian dollars. Setting up an equation we get , which solves to , and the sum of digits of isSolution 2Each time Isabelle exchanges U.S. dollars, she gets Canadian dollars and Canadian dollars extra. Isabelle received a total of Canadian dollars extra, therefore she exchanged U.S. dollars times. Thus .Problem 8Minneapolis-St. Paul International Airport is 8 miles southwest of downtown St. Paul and 10 miles southeast of downtown Minneapolis. Which of the following is closest to the number of miles between downtown St. Paul and downtown Minneapolis?SolutionThe directions "southwest" and "southeast" are orthogonal. Thus the described situation is a right triangle with legs 8 miles and 10 miles long. The hypotenuse length is , and thus the answeris .Without a calculator one can note that . Problem 9A square has sides of length 10, and a circle centered at one of its vertices has radius 10. What is the area of the union of the regions enclosed by the square and the circle?SolutionThe area of the circle is , the area of the square is .Exactly of the circle lies inside the square. Thus the total area is.Problem 10A grocer makes a display of cans in which the top row has one can and each lower row has two more cans than the row above it. If the display contains cans, how many rows does it contain?SolutionThe sum of the first odd numbers is . As in our case , we have .Problem 11Two eight-sided dice each have faces numbered 1 through 8. When the dice are rolled, each face has an equal probability of appearing on the top. What is the probability that the product of the two top numbers is greater than their sum?SolutionSolution 1We have , hence if at least one of the numbers is , the sum is larger. There such possibilities.We have .For we already have , hence all other cases are good.Out of the possible cases, we found that in the sum is greater than or equal to the product, hence in it is smaller. Therefore the answer is .Solution 2Let the two rolls be , and .From the restriction:Since and are non-negative integers between and , either , , orif and only if or .There are ordered pairs with , ordered pairs with , and ordered pair with and . So, there areordered pairs such that .if and only if and or equivalently and . This gives ordered pair .So, there are a total of ordered pairs with .Since there are a total of ordered pairs , there are ordered pairs with .Thus, the desired probability is .Problem 12An annulus is the region between two concentric circles. The concentric circles in the ﬁgure have radii and , with . Let be a radius of the larger circle, let be tangent to the smaller circle at , and let be the radius of the larger circle that contains . Let , , and . What is the area of the annulus?SolutionThe area of the large circle is , the area of the small one is , hence the shaded area is .From the Pythagorean Theorem for the right triangle we have , hence and thus the shaded area is . Problem 13In the United States, coins have the following thicknesses: penny, mm; nickel, mm; dime, mm; quarter, mm. If a stack of these coins is exactly mm high, how many coins are in the stack?SolutionAll numbers in this solution will be in hundreds of a millimeter.The thinnest coin is the dime, with thickness . A stack of dimes has height .The other three coin types have thicknesses , , and . By replacing some of the dimes in our stack by other, thicker coins, we can clearly create exactly all heights in the set.If we take an odd , then all the possible heights will be odd, and thus none of them will be . Hence is even.If the stack will be too low and if it will be too high. Thus we are left with cases and .If the possible stack heights are , with the remaining ones exceeding .Therefore there are coins in the stack.Using the above observation we can easily construct such a stack. A stack of dimes would have height , thus we need to add . This can be done for example by replacing five dimes by nickels (for ), and one dime by a penny (for ).Problem 14A bag initially contains red marbles and blue marbles only, with moreblue than red. Red marbles are added to the bag until only of the marbles in the bag are blue. Then yellow marbles are added to the baguntil only of the marbles in the bag are blue. Finally, the number of blue marbles in the bag is doubled. What fraction of the marbles now in the bag are blue?SolutionWe can ignore most of the problem statement. The only important information is that immediately before the last step blue marbles formed of the marbles in the bag. This means that there were blue and other marbles, for some . When we double the number ofblue marbles, there will be blue and other marbles, hence blue marbles now form of all marbles in the bag.Problem 15Patty has coins consisting of nickels and dimes. If her nickels were dimes and her dimes were nickels, she would have cents more. How much are her coins worth?SolutionSolution 1She has nickels and dimes. Their total cost iscents. If the dimes were nickels and vice versa, she would havecents. This value should be cents more than the previous one. We get , which solves to . Her coins are worth .Solution 2Changing a nickel into a dime increases the sum by cents, and changing a dime into a nickel decreases it by the same amount. As the sum increased by cents, there are more nickels than dimes. As the total count is , this means that there are nickels and dimes.Problem 16Three circles of radius are externally tangent to each other and internally tangent to a larger circle. What is the radius of the large circle?SolutionThe situation in shown in the picture below. The radius we seek is . Clearly . The point is clearly the center of the equilateral triangle , thus is of the altitude of this triangle. We get that . Therefore the radius we seek is.WARNING. Note that the answer does not correspond to any of the five options. Most probably there is a typo in option D.Problem 17The two digits in Jack's age are the same as the digits in Bill's age, but in reverse order. In five years Jack will be twice as old as Bill will be then. What is the difference in their current ages?SolutionSolution 1If Jack's current age is , then Bill's current age is .In five years, Jack's age will be and Bill's age will be .We are given that . Thus .For we get . For and the value is not an integer, and for it is more than . Thus the only solution is , and the difference in ages is .Solution 2Age difference does not change in time. Thus in five years Bill's age will be equal to their age difference.The age difference is , hence it is a multiple of . Thus Bill's current age modulo must be .Thus Bill's age is in the set .As Jack is older, we only need to consider the cases where the tens digit of Bill's age is smaller than the ones digit. This leaves us with the options .Checking each of them, we see that only works, and gives the solution .Problem 18In the right triangle , we have , , and . Points , , and are located on , , and , respectively, so that , , and . What is the ratio of the area of to that of ?SolutionFirst of all, note that , and therefore.Draw the height from onto as in the picture below:Now consider the area of . Clearly the triangles and are similar, as they have all angles equal. Their ratio is ,hence . Now the area of can be computed as= . Similarly we can find that as well.Hence , and the answer is .Problem 19In the sequence , , , , each term after the third is found by subtracting the previous term from the sum of the two terms that precede that term. For example, the fourth term is. What is the term in this sequence?SolutionSolution 1We already know that , , , and . Let's compute the next few terms to get the idea how the sequence behaves. We get ,, , and so on.We can now discover the following pattern: and . This is easily proved by induction. It follows that.Solution 2Note that the recurrence can be rewritten as.Hence we get that and also From the values given in the problem statement we see that .From we get that .From we get that .Following this pattern, we get.Problem 20In points and lie on and , respectively. If and intersect at so that and , what isSolutionSolution (Triangle Areas)We use the square bracket notation to denote area.Without loss of generality, we can assume . Then , and . We have , so we need to find the area of quadrilateral .Draw the line segment to form the two triangles and . Let , and . By considering trianglesand , we obtain , and by considering triangles and , we obtain . Solving, we get , , so the area of quadrilateral is .ThereforeSolution (Mass points)The presence of only ratios in the problem essentially cries out for mass points.As per the problem, we assign a mass of to point , and a mass of to . Then, to balance and on , has a mass of .Now, were we to assign a mass of to and a mass of to , we'd have . Scaling this down by (to get , which puts and in terms of the masses of and ), we assign a mass of to and a mass of to .Now, to balance and on , we must give a mass of . Finally, the ratio of to is given by the ratio of the mass of tothe mass of , which is .Solution (Coordinates)Affine transformations preserve ratios of distances, and for any pair of triangles there is an affine transformation that maps the first one onto the second one. This is why the answer is the same for any , and we just need to compute it for any single triangle.We can choose the points , , and . This way we will have , and . The situation is shown in the picture below:The point is the intersection of the lines and . The points on the first line have the form , the points on the second line have the form . Solving for we get , hence.The ratio can now be computed simply by observing the coordinates of , , and :Problem 21Let ; ; and ; ; be two arithmetic progressions. The set is the union of the first terms of each sequence. How many distinct numbers are in ?SolutionThe two sets of terms are and.Now . We can compute. We will now find .Consider the numbers in . We want to find out how many of them lie in . In other words, we need to find out the number of valid values of for which .The fact "" can be rewritten as ", and ".The first condition gives , the second one gives .Thus the good values of are , and their count is .Therefore , and thus .Problem 22A triangle with sides of 5, 12, and 13 has both an inscribed and a circumscribed circle. What is the distance between the centers of those circles?SolutionThis is obviously a right triangle. Pick a coordinate system so that the right angle is at and the other two vertices are at and .As this is a right triangle, the center of the circumcircle is in the middle of the hypotenuse, at .The radius of the inscribed circle can be computed using the well-known identity , where is the area of the triangle and its perimeter. In our case, and , thus . As the inscribed circle touches both legs, its center must be at .The distance of these two points is then.Problem 23Each face of a cube is painted either red or blue, each with probability 1/2. The color of each face is determined independently. What is the probability that the painted cube can be placed on a horizontal surface so that the four vertical faces are all the same color?SolutionLabel the six sides of the cube by numbers to as on a classic dice. Then the "four vertical faces" can be: , , or .Let be the set of colorings where are all of the same color, similarly let and be the sets of good colorings for the other two sets of faces.There are possible colorings, and there are goodcolorings. Thus the result is . We need to compute .Using the Principle of Inclusion-Exclusion we can writeClearly , as we have two possibilities for the common color of the four vertical faces, and two possibilities for each of the horizontal faces.What is ? The faces must have the same color, and at the same time faces must have the same color. It turns out thatthe set containing just the two cubes where all six faces have the same color.Therefore , and the result is .Problem 24In we have , , and . Point is on the circumscribed circle of the triangle so that bisects . What is the value of ?SolutionProblem 25A circle of radius is internally tangent to two circles of radius at points and , where is a diameter of the smaller circle. What is the area of the region, shaded in the picture, that is outside the smaller circle and inside each of the two larger circles?SolutionThe area of the small circle is . We can add it to the shaded region, compute the area of the new region, and then subtract the area of the small circle from the result.Let and be the intersections of the two large circles. Connect them to and to get the picture below:Now obviously the triangles and are equilateral with side .Take a look at the bottom circle. The angle is , hence the sector is of the circle. The same is true for the sector of the bottom circle, and sectors and of the top circle.If we now sum the areas of these four sectors, we will almost get the area of the new shaded region - except that each of the two equilateral triangles will be counted twice.Hence the area of the new shaded region is, and the area of the original shared region is .- 21 -。

AMC 美国数学竞赛 2000 AMC 10 试题及答案解析

USA AMC 10 20001In the year , the United States will host the International Mathematical Olympiad. Let , , and be distinct positive integers such that the product . What's the largest possible value of the sum ?SolutionThe sum is the highest if two factors are the lowest.So, and .2Solution.3Each day, Jenny ate of the jellybeans that were in her jar at the beginning of the day. At the end of the second day, remained. How many jellybeans were in the jar originally?Solution4Chandra pays an online service provider a fixed monthly fee plus an hourly charge for connect time. Her December bill was , but in January her bill was because she used twice as much connect time as in December. What is the fixxed monthly fee?SolutionLet be the fixed fee, and be the amount she pays for the minutes she used in the first month.We want the fixed fee, which is5Points and are the midpoints of sides and of . As moves along a line that is parallel to side , how many of the four quantities listed below change?(a) the length of the segment(b) the perimeter of(c) the area of(d) the area of trapezoidSolution(a) Clearly does not change, and , so doesn't change either.(b) Obviously, the perimeter changes.(c) The area clearly doesn't change, as both the base and its corresponding height remain the same.(d) The bases and do not change, and neither does the height, so the trapezoid remains the same.Only quantity changes, so the correct answer is .6The Fibonacci Sequence starts with two 1s and each term afterwards is the sum of its predecessors. Which one of the ten digits is the last to appear in thet units position of a number in the Fibonacci Sequence?SolutionThe pattern of the units digits areIn order of appearance:.is the last.7In rectangle , , is on , and and trisect . What is the perimeter of ?Solution.Since is trisected, .Thus,.Adding, .8At Olympic High School, of the freshmen and of the sophomores took the AMC-10. Given that the number of freshmen and sophomore contestants was the same, which of the following must be true?There are five times as many sophomores as freshmen.There are twice as many sophomores as freshmen.There are as many freshmen as sophomores.There are twice as many freshmen as sophomores.There are five times as many freshmen as sophomores.SolutionLet be the number of freshman and be the number of sophomores.There are twice as many freshmen as sophomores.9If , where , thenSolution, so ...10The sides of a triangle with positive area have lengths , , and . The sides of a second triangle with positive area have lengths , , and . What is the smallest positive number that is not a possible value of ?SolutionFrom the triangle inequality, and . The smallest positive number not possible is , which is .11Two different prime numbers between and are chosen. When their sum is subtracted from their product, which of the following numbers could be obtained?SolutionTwo prime numbers between and are both odd.Thus, we can discard the even choices.Both and are even, so one more than is a multiple of four.is the only possible choice.satisfy this, .12Figures , , , and consist of , , , and nonoverlapping unit squares, respectively. If the pattern were continued, how many nonoverlapping unit squares would there be in figure 100?SolutionSolution 1We have a recursion:.I.E. we add increasing multiples of each time we go up a figure. So, to go from Figure 0 to 100, we add.Solution 2We can divide up figure to get the sum of the sum of the firstodd numbers and the sum of the first odd numbers. If you do not see this, here is the example for :The sum of the first odd numbers is , so for figure , there are unit squares. We plug in to get , which is choice13There are 5 yellow pegs, 4 red pegs, 3 green pegs, 2 blue pegs, and 1 orange peg to be placed on a triangular peg board. In how many ways can the pegs be placed so that no (horizontal) row or (vertical) column contains two pegs of the same color?SolutionIn each column there must be one yellow peg. In particular, in the rightmost column, there is only one peg spot, therefore a yellow peg must go there.In the second column from the right, there are two spaces for pegs. One of them is in the same row as the corner peg, so there is only one remaining choice left for the yellow peg in this column.By similar logic, we can fill in the yellow pegs as shown:After this we can proceed to fill in the whole pegboard, so there is only arrangement of the pegs. The answer is14Mrs. Walter gave an exam in a mathematics class of five students. She entered the scores in random order into a spreadsheet, which recalculated the class average after each score was entered. Mrs. Walter noticed that after each score was entered, the average was always an integer. The scores (listed in ascending order) were , , , , and . What was the last score Mrs. Walter entered? SolutionThe sum of the first scores must be even, so we must choose evens or the odds to be the first two scores.Let us look at the numbers in mod .If we choose the two odds, the next number must be a multiple of , of which there is none.Similarly, if we choose or , the next number must be a multiple of , of which there is none.So we choose first.The next number must be 1 in mod 3, of which only remains.The sum of the first three scores is . This is equivalent to in mod .Thus, we need to choose one number that is in mod . is the only one that works.Thus, is the last score entered.15Two non-zero real numbers, and , satisfy . Which of the following is a possible value of ?SolutionSubstituting , we get16The diagram shows lattice points, each one unit from its nearest neighbors. Segment meets segment at . Find the length of segment .SolutionSolution 1Let be the line containing and and let be the line containing and . If we set the bottom left point at , then , , , and .The line is given by the equation . The -intercept is , so . We are given two points on , hence we cancompute the slope, to be , so is the lineSimilarly, is given by . The slope in this case is , so . Plugging in the point gives us , so is the line .At , the intersection point, both of the equations must be true, soWe have the coordinates of and , so we can use the distance formula here:which is answer choiceSolution 2Draw the perpendiculars from and to , respectively. As it turns out, . Let be the point on for which ., and , so by AA similarity,By the Pythagorean Theorem, we have ,, and . Let , so , thenThis is answer choiceAlso, you could extend CD to the end of the box and create two similar triangles. Then use ratios and find that the distance is 5/9 of the diagonal AB. Thus, the answer is B.17Boris has an incredible coin changing machine. When he puts in a quarter, it returns five nickels; when he puts in a nickel, it returns five pennies; and when he puts in a penny, it returns five quarters. Boris starts with just one penny. Which of the following amounts could Boris have after using the machine repeatedly?SolutionConsider what happens each time he puts a coin in. If he puts in a quarter, he gets five nickels back, so the amount of money he has doesn't change. Similarly, if he puts a nickel in the machine, he gets five pennies back and the money value doesn't change. However, if he puts a penny in, he gets five quarters back, increasing the amount of money he has by cents.This implies that the only possible values, in cents, he can have are the ones one more than a multiple of . Of the choices given, the only one is18Charlyn walks completely around the boundary of a square whose sides are each km long. From any point on her path she can see exactly km horizontally in all directions. What is the area of the region consisting of all points Charlyn can see during her walk, expressed in square kilometers and rounded to the nearest whole number?SolutionThe area she sees looks at follows:The part inside the walk has area . The part outside the walk consists of four rectangles, and four arcs. Each of the rectangles has area . The four arcs together form a circle with radius . Therefore the total area she can see is, which rounded to the nearest integer is .19Through a point on the hypotenuse of a right triangle, lines are drawn parallel to the legs of the triangle so that the trangle is divided into a square and two smaller right triangles. The area of one of the two small right triangles is times the area of the square. The ratio of the area of the other small right triangle to the area of the square is SolutionLet the square have area , then it follows that the altitude of one of the triangles is . The area of the other triangle is .By similar triangles, we haveThis is choice(Note that this approach is enough to get the correct answer in the contest. However, if we wanted a completely correct solution, we should also note that scaling the given triangle times changes each of the areas times, and therefore it does not influence the ratio of any two areas. This is why we can pick the side of the square.)20Let , , and be nonnegative integers such that . What is the maximum value of ? SolutionThe trick is to realize that the sum is similar to the product .If we multiply , we get.We know that , therefore.Therefore the maximum value of is equal to the maximum value of . Now we will find this maximum.Suppose that some two of , , and differ by at least . Then this triple is surely not optimal.Proof: WLOG let . We can then increase the value ofby changing and .Therefore the maximum is achieved in the cases where is a rotation of . The value of in this case is . And thus the maximum of is.21If all alligators are ferocious creatures and some creepy crawlers are alligators, which statement(s) must be true?I. All alligators are creepy crawlers.II. Some ferocious creatures are creepy crawlers.III. Some alligators are not creepy crawlers.SolutionWe interpret the problem statement as a query about three abstract concepts denoted as "alligators", "creepy crawlers" and "ferocious creatures". In answering the question, we may NOT refer to reality -- for example to the fact that alligators do exist.To make more clear that we are not using anything outside the problem statement, let's rename the three concepts as , , and .We got the following information:▪If is an , then is an .▪There is some that is a and at the same time an .We CAN NOT conclude that the first statement is true. For example, the situation "Johnny and Freddy are s, but only Johnny is a "meets both conditions, but the first statement is false.We CAN conclude that the second statement is true. We know that there is some that is a and at the same time an . Pick one such and call it Bobby. Additionally, we know that if is an , then is an. Bobby is an , therefore Bobby is an . And this is enough to prove the second statement -- Bobby is an that is also a .We CAN NOT conclude that the third statement is true. For example, consider the situation when , and are equivalent (represent the same set of objects). In such case both conditions are satisfied, but the third statement is false.Therefore the answer is .22One morning each member of Angela's family drank an -ounce mixture of coffee with milk. The amounts of coffee and milk varied from cup to cup, but were never zero. Angela drank a quarter of the total amount of milk and a sixth of the total amount of coffee. How many people are in the family?SolutionThe exact value "8 ounces" is not important. We will only use the fact that each member of the family drank the same amount.Let be the total number of ounces of milk drank by the family and the total number of ounces of coffee. Thus the whole family drank a total of ounces of fluids.Let be the number of family members. Then each family member drank ounces of fluids.We know that Angela drank ounces of fluids.As Angela is a family member, we have .Multiply both sides by to get .If , we have .If , we have .Therefore the only remaining option is .23When the mean, median, and mode of the list are arranged in increasing order, they form a non-constant arithmetic progression. What is the sum of all possible real values of ? SolutionAs occurs three times and each of the three other values just once, regardless of what we choose the mode will always be .The sum of all numbers is , therefore the mean is .The six known values, in sorted order, are . From this sequence we conclude: If , the median will be . If , the median will be . Finally, if , the median will be .We will now examine each of these three cases separately.In the case , both the median and the mode are 2, therefore we can not get any non-constant arithmetic progression.In the case we have , because. Therefore our three values inorder are . We want this to be an arithmetic progression. From the first two terms the difference must be . Therefore thethird term must be .Solving we get the only solution for this case: . The case remains. Once again, we have ,therefore the order is . The only solution is when , i. e., .The sum of all solutions is therefore .24Let be a function for which . Find the sum of all values of for which .SolutionIn the definition of , let . We get: . As we have , we must have , in other words .One can now either explicitly compute the roots, or use Vieta's formulas. According to them, the sum of the roots ofis . In our case this is .(Note that for the above approach to be completely correct, we should additionally verify that there actually are two distinct real roots. This is, for example, obvious from the facts that and .)25In year , the day of the year is a Tuesday. In year , the day is also a Tuesday. On what day of the week did the of year occur?SolutionClearly, identifying what of these years may/must/may not be a leap year will be key in solving the problem.Let be the day of year , the day of year and the day of year .If year is not a leap year, the day will bedays after . As , that would be a Monday.Therefore year must be a leap year. (Then is days after .) As there can not be two leap years after each other, is not a leap year. Therefore day is days after . We have . Therefore is weekdays before , i.e., is a.(Note that the situation described by the problem statement indeed occurs in our calendar. For example, for we have=Tuesday, October 26th 2004, =Tuesday, July 19th, 2005 and =Thursday, April 10th 2003.)。

2023年AMC10美国数学竞赛A卷附中文翻译和答案

2023AMC10美国数学竞赛A卷1. A cell phone plan costs $20 each month, plus 5¢ per text message sent, plus 10¢ for each minute used over 30 hours. In January Michelle sent 100 text messages and talked for 30.5 hours. How much did she have to pay?(A) $24.00 (B) $24.50 (C) $25.50 (D) $28.00 (E) $30.002. A small bottle of shampoo can hold 35 milliliters of shampoo, Whereas a large bottle can hold 500 milliliters of shampoo. Jasmine wants to buy the minimum number of small bottles necessary to completely fill a large bottle. How many bottles must she buy?(A) 11 (B) 12 (C) 13 (D) 14 (E) 153. Suppose [a b] denotes the average of a and b, and {a b c} denotes the average of a, b, and c. What is {{1 1 0} [0 1] 0}?(A) (B)(C)(D) (E)4. Let X and Y be the following sums of arithmetic sequences:X= 10 + 12 + 14 + …+ 100.Y= 12 + 14 + 16 + …+ 102.What is the value of(A) 92 (B) 98 (C) 100 (D) 102 (E) 1125. At an elementary school, the students in third grade, fourth grade, and fifth grade run an average of 12, 15, and 10 minutes per day, respectively. There are twice as many third graders as fourth graders, and twice as many fourth graders as fifth graders. What is the average number of minutes run per day by these students?(A) 12 (B) (C) (D) 13 (E) 146. Set A has 20 elements, and set B has 15 elements. What is the smallest possible number of elements in A∪B, the union of A and B?(A) 5 (B) 15 (C) 20 (D) 35 (E) 3007. Which of the following equations does NOT have a solution?(A) (B) (C)(D) (E)8. Last summer 30% of the birds living on Town Lake were geese, 25% were swans, 10% were herons, and 35% were ducks. What percent of the birds that were not swans were geese?(A) 20 (B) 30 (C) 40 (D) 50 (E) 609. A rectangular region is bounded by the graphs of the equations y=a, y=-b, x=-c, and x=d, where a, b, c, and d are all positive numbers. Which of the following represents the area of this region?(A) ac + ad + bc + bd (B) ac – ad + bc – bd (C) ac + ad – bc – bd(D) –ac –ad + bc + bd (E) ac – ad – bc + bd10. A majority of the 20 students in Ms. Deameanor’s class bought pencils at the school bookstore. Each of these students bought the same number of pencils, and this number was greater than 1. The cost of a pencil in cents was greater than the number of pencils each student bought, and the total cost of all the pencils was $17.71. What was the cost of a pencil in cents?(A) 7 (B) 11 (C) 17 (D) 23 (E) 7711. Square EFGH has one vertex on each side of square ABCD. Point E is on AB with AE=7·EB. What is the ratio of the area of EFGH to the area of ABCD?(A) (B)(C)(D) (E)12. The players on a basketball team made some three-point shots, some two-point shots, some one-point free throws. They scored as many points with two-point shots as with three-point shots. Their number of successful free throws was one more than their number of successful two-point shots. The team’s total score was 61 points. How many free throws did they make?(A) 13 (B) 14 (C) 15 (D) 16 (E) 1713. How many even integers are there between 200 and 700 whose digits are alldifferent and come from the set {1, 2, 5, 7, 8, 9}?(A) 12 (B)20 (C)72 (D) 120 (E) 20014. A pair of standard 6-sided fair dice is rolled once. The sum of the numbers rolled determines the diameter of a circle. What is the probability that the numerical value of the area of the circle is less than the numerical value of the circle’s circumference?(A) (B)(C)(D) (E)15. Roy bought a new battery-gasoline hybrid car. On a trip the car ran exclusively on its battery for the first 40 miles, then ran exclusively on gasoline for the rest of the trip, using gasoline at a rate of 0.02 gallons per mile. On the whole trip he averaged55 miles per gallon. How long was the trip in miles?(A) 140 (B) 240 (C) 440 (D) 640 (E) 84016. Which of the following in equal to(A) (B) (C) (D) (E)17. In the eight-term sequence A, B, C, D, E, F, G, H, the value of C is 5 and the sum of any three consecutive terms is 30. What is A + H?(A) 17 (B) 18 (C) 25 (D) 26 (E) 4318. Circles A, B, and C each have radius 1. Circles A and B share one point of tangency. Circle C has a point of tangency with the midpoint of AB. What is the area inside Circle C but outside Circle A and Circle B?(B) (C) (D) (E)(A)19. In 1991 the population of a town was a perfect square. Ten years later, after an increase of 150 people, the population was 9 more than a perfect square. Now, in 2023, with an increase of another 150 people, the population is once again a perfect square. Which of the following is closest to the percent growth of the town’s popu lation during this twenty-year period?(A) 42 (B) 47 (C) 52 (D) 57 (E) 6220. Two points on the circumference of a circle of radius r are selected independently and at random. From each point a chord of length r is drawn in a clockwise direction. What is the probability that the two chords intersect?(A) (B) (C) (D) (E)21. Two counterfeit coins of equal weight are mixed with 8 identical genuine coins. The weight of each of the counterfeit coins is different from the weight of each of the genuine coins. A pair of coins is selected at random without replacement from the 10 coins. A second pair is selected at random without replacement from the remaining 8coins. The combined weight of the first pair is equal to the combined weight of the second pair. What is the probability that all 4 selected coins are genuine?(A) (B) (C) (D) (E)22. Each vertex of convex pentagon ABCDE is to be assigned a color. There are 6 colors to choose from, and the ends of each diagonal must have different colors. How many different colorings are possible?(A) 2500 (B) 2880 (C) 3120 (D) 3250 (E) 375023. Seven students count from 1 to 1000 as follows:·Alice says all the numbers, except she skips the middle number in each consecutive group of thre e numbers. That is Alice says 1, 3, 4, 6, 7, 9, …, 997, 999, 1000.·Barbara says all of the numbers that Alice doesn’t say, except she also skips the middle number in each consecutive grope of three numbers.·Candice says all of the numbers that neither Alice nor Barbara says, except she also skips the middle number in each consecutive group of three numbers. ·Debbie, Eliza, and Fatima say all of the numbers that none of the students with the first names beginning before theirs in the alphabet say, except each also skips the middle number in each of her consecutive groups of three numbers.·Finally, George says the only number that no one else says.What number does George say?(A) 37 (B) 242 (C) 365 (D) 728 (E) 99824. Two distinct regular tetrahedra have all their vertices among the vertices of the same unit cube. What is the volume of the region formed by the intersection of the tetrahedra?(A) (B) (C) (D) (E)an integer. A point X in the interior of R is25. Let R be a square region andcalled n-ray partitional if there are n rays emanating from X that divide R into N triangles of equal area. How many points are 100-ray partitional but not 60-ray partitional?(A) 1500 (B) 1560 (C) 2320 (D) 2480 (E) 25002023AMC10美国数学竞赛A卷1. 某通讯公司手机每月基本费为20美元, 每传送一则简讯收 5美分（一美元=100 美分）。

AMC8(美国数学竞赛)历年真题、答案及中英文解析

AMC8（美国数学竞赛）历年真题、答案及中英文解析艾蕾特教育的AMC8 美国数学竞赛考试历年真题、答案及中英文解析：AMC8-2020年：真题 --- 答案---解析（英文解析+中文解析）AMC8 - 2019年：真题----答案----解析（英文解析+中文解析）AMC8 - 2018年：真题----答案----解析（英文解析+中文解析）AMC8 - 2017年：真题----答案----解析（英文解析+中文解析）AMC8 - 2016年：真题----答案----解析（英文解析+中文解析）AMC8 - 2015年：真题----答案----解析（英文解析+中文解析）AMC8 - 2014年：真题----答案----解析（英文解析+中文解析）AMC8 - 2013年：真题----答案----解析（英文解析+中文解析）AMC8 - 2012年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 2010年：真题----答案----解析（英文解析+中文解析）AMC8 - 2009年：真题----答案----解析（英文解析+中文解析）AMC8 - 2008年：真题----答案----解析（英文解析+中文解析）AMC8 - 2007年：真题----答案----解析（英文解析+中文解析）AMC8 - 2006年：真题----答案----解析（英文解析+中文解析）AMC8 - 2005年：真题----答案----解析（英文解析+中文解析）AMC8 - 2004年：真题----答案----解析（英文解析+中文解析）AMC8 - 2003年：真题----答案----解析（英文解析+中文解析）AMC8 - 2002年：真题----答案----解析（英文解析+中文解析）AMC8 - 2001年：真题----答案----解析（英文解析+中文解析）AMC8 - 2000年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1998年：真题----答案----解析（英文解析+中文解析）AMC8 - 1997年：真题----答案----解析（英文解析+中文解析）AMC8 - 1996年：真题----答案----解析（英文解析+中文解析）AMC8 - 1995年：真题----答案----解析（英文解析+中文解析）AMC8 - 1994年：真题----答案----解析（英文解析+中文解析）AMC8 - 1993年：真题----答案----解析（英文解析+中文解析）AMC8 - 1992年：真题----答案----解析（英文解析+中文解析）AMC8 - 1991年：真题----答案----解析（英文解析+中文解析）AMC8 - 1990年：真题----答案----解析（英文解析+中文解析）AMC8 - 1989年：真题----答案----解析（英文解析+中文解析）AMC8 - 1988年：真题----答案----解析（英文解析+中文解析）析）AMC8 - 1986年：真题----答案----解析（英文解析+中文解析）AMC8 - 1985年：真题----答案----解析（英文解析+中文解析）◆AMC介绍◆AMC（American Mathematics Competitions) 由美国数学协会（MAA）组织的数学竞赛，分为 AMC8 、 AMC10、 AMC12 。

AMC 美国数学竞赛 2001 AMC 10 试题及答案解析

USA AMC 10 20011The median of the listis . What is the mean?Solution2A number is more than the product of its reciprocal and its additive inverse. In which interval does the number lie?Solution3The sum of two numbers is . Suppose 3 is added to each number and then each of the resulting numbers is doubled. What is the sum of the final two numbers?Solution4What is the maximum number of possible points of intersection of a circle and a triangle?Solution5How many of the twelve pentominoes pictured below have at least one line of symettry?Solution6Let and denote the product and the sum, respectively, of thedigits of the integer . For example, and . Supposeis a two-digit number such that . What is the units digit of ?Solution7When the decimal point of a certain positive decimal number is moved four places to the right, the new number is four times the reciprocal of the original number. What is the original number?Solution8Wanda, Darren, Beatrice, and Chi are tutors in the school math lab. Their schedule is as follows: Darren works every third school day, Wanda works every fourth school day, Beatrice works every sixth school day, and Chi works every seventh school day. Today they are all working in the math lab. In how many school days from today willthey next be together tutoring in the lab?Solution9The state income tax where Kristin lives is levied at the rate of of the first of annual income plus of any amount above . Kristin noticed that the state income tax she paid amounted to of her annual income. What was her annual income?Solution10If , , and are positive with , , and , then isSolution11Consider the dark square in an array of unit squares, part of which is shown. The ﬁrst ring of squ ares around this center square contains unit squares. The second ring contains unit squares. If we continue this process, the number of unit squares in the ring isSolution12Suppose that is the product of three consecutive integers and that is divisible by . Which of the following is not necessarily a divisor of Solution13A telephone number has the form , where each letter represents a different digit. The digits in each part of the numbers are in decreasing order; that is, , , and . Furthermore, , , and are consecutive even digits; , , , and are consecutive odd digits; and . Find .Solution14A charity sells 140 benefit tickets for a total of . Some tickets sell for full price (a whole dollar amount), and the rest sells for half price. How much money is raised by the full-price tickets?Solution15A street has parallel curbs feet apart. A crosswalk bounded by two parallel stripes crosses the street at an angle. The length of the curb between the stripes is feet and each stripe is feet long. Find the distance, in feet, between the stripes.Solution16The mean of three numbers is 10 more than the least of the numbers and 15 less than the greatest. The median of the three numbers is 5. What is their sum?Solution17Which of the cones listed below can be formed from a sector of a circle of radius by aligning the two straight sides?A cone with slant height of and radiusA cone with height of and radiusA cone with slant height of and radiusA cone with height of and radiusA cone with slant height of and radiusSolution18The plane is tiled by congruent squares and congruent pentagons as indicated. The percent of the plane that is enclosed by the pentagons is closest toSolution19Pat wants to buy four donuts from an ample supply of three types of donuts: glazed, chocolate, and powdered. How many different selections are possible?Solution20A regular octagon is formed by cutting an isosceles right triangle from each of the corners of a square with sides of length . What is the length of each side of the octagon?Solution21A right circular cylinder with its diameter equal to its height is inscribed in a right circular cone. The cone has diameter and altitude , and the axes of the cylinder and cone coincide. Find the radius of the cylinder.Solution22In the magic square shown, the sums of the numbers in each row, column, and diagonal are the same. Five of these numbers are represented by , , , , and . Find .Solution23A box contains exactly five chips, three red and two white. Chips are randomly removed one at a time without replacement until all the red chips are drawn or all the white chips are drawn. What is the probability that the last chip drawn is white?Solution24In trapezoid , and are perpendicular to , with, , and . What is ?Solution25How many positive integers not exceeding are multiples of or but not ?。

AMC 美国数学竞赛 2002 AMC 10A 试题及答案解析

2002 AMC 10A1、The ratio is closest to which of the following numbers?SolutionWe factor as . As , ouranswer is .2、For the nonzero numbers , , , define.Find .Solution. Ouranswer is then .Alternate solution for the lazy: Without computing the answer exactly,we see that , , and . The sumis , and as all the options are integers, the correct one is obviously .3、According to the standard convention for exponentiation,.If the order in which the exponentiations are performed is changed, how many other values are possible?SolutionThe best way to solve this problem is by simple brute force.It is convenient to drop the usual way how exponentiation is denoted,and to write the formula as , where denotes exponentiation. We are now examining all ways to add parentheses to this expression. There are 5 ways to do so:1.2.3.4.5.We can note that . Therefore options 1 and 2 are equal, and options 3 and 4 are equal. Option 1 is the one given in the problem statement. Thus we only need to evaluate options 3 and 5.Thus the only other result is , and our answer is .4、For how many positive integers does there exist at least one positive integer such that ?infinitely manySolutionSolution 1For any we can pick , we get , therefore theanswer is .Solution 2Another solution, slightly similar to this first one would be using Simon's Favorite Factoring Trick.Let , thenThis means that there are infinitely many numbers that can satisfythe inequality. So the answer is .5、Each of the small circles in the figure has radius one. The innermost circle is tangent to the six circles that surround it, and each of those circles is tangent to the large circle and to its small-circle neighbors. Find the area of the shaded region.SolutionThe outer circle has radius , and thus area . The littlecircles have area each; since there are 7, their total area is . Thus,our answer is .6、Cindy was asked by her teacher to subtract from a certain numberand then divide the result by . Instead, she subtracted and thendivided the result by , giving an answer of . What would heranswer have been had she worked the problem correctly?SolutionWe work backwards; the number that Cindy started with is. Now, the correct result is . Ouranswer is .7、If an arc of on circle has the same length as an arc of oncircle , then the ratio of the area of circle to the area of circle isSolutionLet and be the radii of circles A and B, respectively.It is well known that in a circle with radius r, a subtended arc oppositean angle of degrees has length .Using that here, the arc of circle A has length . The arcof circle B has length . We know that they are equal,so , so we multiply through and simplify to get . As all circles are similar to one another, the ratio of the areas is just thesquare of the ratios of the radii, so our answer is .8、Betsy designed a flag using blue triangles, small white squares, anda red center square, as shown. Let be the total area of the bluetriangles, the total area of the white squares, and the area of thered square. Which of the following is correct?SolutionThe blue that's touching the center red square makes up 8 triangles, or 4 squares. Each of the corners is 2 squares and each of the edges is 1, totaling 12 squares. There are 12 white squares, thus we have.9、There are 3 numbers A, B, and C, such that ,and . What is the average of A, B, and C?More than 1SolutionNotice that we don't need to find what A, B, and C actually are, just their average. In other words, if we can find A+B+C, we will be done.Adding up the equations gives soand the average is . Our answer is .10、Compute the sum of all the roots of.SolutionSolution 1We expand to get which isafter combining like terms. Using the quadratic partof Vieta's Formulas, we find the sum of the roots is . Solution 2Combine terms to get, hence the rootsare and , thus our answer is .11、Jamal wants to store computer files on floppy disks, each ofwhich has a capacity of megabytes (MB). Three of his files requireMB of memory each, more require MB each, and theremaining require MB each. No file can be split between floppydisks. What is the minimal number of floppy disks that will hold all the files?SolutionA 0.8 MB file can either be on its own disk, or share it with a 0.4 MB. Clearly it is not worse to pick the second possibility. Thus we will have 3 disks, each with one 0.8 MB file and one 0.4 MB file.We are left with 12 files of 0.7 MB each, and 12 files of 0.4 MB each.Their total size is MB. The total capacity of 9 disks is MB, hence we need at least 10 more disks. And wecan easily verify that 10 disks are indeed enough: six of them will carry two 0.7 MB files each, and four will carry three 0.4 MB files each.Thus our answer is .12、Mr. Earl E. Bird leaves his house for work at exactly 8:00 A.M. every morning. When he averages miles per hour, he arrives at hisworkplace three minutes late. When he averages miles per hour, hearrives three minutes early. At what average speed, in miles per hour, should Mr. Bird drive to arrive at his workplace precisely on time?SolutionSolution 1Let the time he needs to get there in be t and the distance he travelsbe d. From the given equations, we know that and. Setting the two equal, we have andwe find of an hour. Substituting t back in, we find . From, we find that r, and our answer, is .Solution 2Since either time he arrives at is 3 minutes from the desired time, the answer is merely the harmonic mean of 40 and 60. The harmonicmean of a and b is . In this case, a and b are 40 and 60,so our answer is , so .Solution 3A more general form of the argument in Solution 2, with proof:Let be the distance to work, and let be the correct average speed.Then the time needed to get to work is .We know that and . Summing these twoequations, we get: .Substituting and dividing both sides by , we get ,hence .(Note that this approach would work even if the time by which he is late was different from the time by which he is early in the other case - we would simply take a weighed sum in step two, and hence obtaina weighed harmonic mean in step three.)13、Give a triangle with side lengths 15, 20, and 25, find the triangle's smallest height.SolutionSolution 1This is a Pythagorean triple (a 3-4-5 actually) with legs 15 and 20. Thearea is then . Now, consider an altitude drawn to anyside. Since the area remains constant, the altitude and side to which it is drawn are inversely proportional. To get the smallest altitude, it must be drawn to the hypotenuse. Let the length be x; we have, so and x is 12. Our answer is then.Solution 2By Heron's formula, the area is , hence the shortest altitude'slength is .14、Both roots of the quadratic equation are prime numbers. The number of possible values of isSolutionConsider a general quadratic with the coefficient of being and theroots being and . It can be factored as which is just. Thus, the sum of the roots is the negative of the coefficient of and the product is the constant term. (In general, this leads to Vieta's Formulas).We now have that the sum of the two roots is while the product is. Since both roots are primes, one must be , otherwise the sumwould be even. That means the other root is and the product mustbe . Hence, our answer is .15、Using the digits 1, 2, 3, 4, 5, 6, 7, and 9, form 4 two-digit prime numbers, using each digit only once. What is the sum of the 4 prime numbers?SolutionOnly odd numbers can finish a two-digit prime number, and a two-digit number ending in 5 is divisible by 5 and thus composite,hence our answer is .(Note that we did not need to actually construct the primes. If we had to, one way to match the tens and ones digits to form four primes is , , , and .)16、Let . What is?SolutionLet . Since one ofthe sums involves a, b, c, and d, it makes sense to consider 4x. We have. Rearranging, we have , so .Thus, our answer is .17、Sarah pours four ounces of coffee into an eight-ounce cup and fourounces of cream into a second cup of the same size. She then transfers half the coffee from the first cup to the second and, after stirring thoroughly, transfers half the liquid in the second cup back to the first. What fraction of the liquid in the first cup is now cream?SolutionWe will simulate the process in steps.In the beginning, we have:▪ounces of coffee in cup▪ounces of cream in cupIn the first step we pour ounces of coffee from cup to cup ,getting:▪ounces of coffee in cup▪ounces of coffee and ounces of cream in cupIn the second step we pour ounce of coffee and ounces of cream from cup to cup , getting:▪ounces of coffee and ounces of cream in cup▪the rest in cupHence at the end we have ounces of liquid in cup , and outof these ounces is cream. Thus the answer is .18、A cube is formed by gluing together 27 standard cubicaldice. (On a standard die, the sum of the numbers on any pair of opposite faces is 7.) The smallest possible sum of all the numbers showing on the surface of the cube isSolutionIn a 3x3x3 cube, there are 8 cubes with three faces showing, 12 with two faces showing and 6 with one face showing. The smallest sum with three faces showing is 1+2+3=6, with two faces showing is 1+2=3, and with one face showing is 1. Hence, the smallest possiblesum is . Our answer is thus.19、Spot's doghouse has a regular hexagonal base that measures oneyard on each side. He is tethered to a vertex with a two-yard rope.What is the area, in square yards, of the region outside of the doghouse that Spot can reach?SolutionPart of what Spot can reach is of a circle with radius 2, whichgives him . He can also reach two parts of a unit circle, whichcombines to give . The total area is then , which gives .20、Points and lie, in that order, on , dividing it intofive segments, each of length 1. Point is not on line . Point lieson , and point lies on . The line segments andare parallel. Find .SolutionAs is parallel to , angles FHD and FGA are congruent. Also,angle F is clearly congruent to itself. From SSS similarity,; hence . Similarly, . Thus,.21、The mean, median, unique mode, and range of a collection of eight integers are all equal to 8. The largest integer that can be an element of this collection isSolutionAs the unique mode is , there are at least two s.As the range is and one of the numbers is , the largest one can beat most .If the largest one is , then the smallest one is , and thus the meanis strictly larger than , which is a contradiction.If the largest one is , then the smallest one is . This means that wealready know four of the values: , , , . Since the mean of all thenumbers is , their sum must be . Thus the sum of the missing fournumbers is . But if is the smallest number,then the sum of the missing numbers must be at least ,which is again a contradiction.If the largest number is , we can easily find the solution. Hence, our answer is .NoteThe solution for is, in fact, unique. As the median must be , thismeans that both the and the number, when ordered by size,must be s. This gives the partial solution . For themean to be each missing variable must be replaced by the smallestallowed value.22、A sit of tiles numbered 1 through 100 is modified repeatedly by the following operation: remove all tiles numbered with a perfect square, and renumber the remaining tiles consecutively starting with 1. How many times must the operation be performed to reduce the number of tiles in the set to one?SolutionSolution 1The pattern is quite simple to see after listing a couple of terms.Solution 2Given tiles, a step removes tiles, leaving tiles behind. Now,, so in the next step tilesare removed. This gives , another perfect square.Thus each two steps we cycle down a perfect square, and insteps, we are left with tile, hence our answer is.23、Points and lie on a line, in that order, with and. Point is not on the line, and . The perimeterof is twice the perimeter of . Find .SolutionFirst, we draw an altitude to BC from E.Let it intersect at M. As triangle BEC is isosceles, we immediately get MB=MC=6, so the altitude is 8. Now, let . Using the Pythagorean Theorem on triangleEMA, we find . From symmetry,as well. Now, we use the fact that the perimeter of is twice the perimeter of .We have so. Squaring both sides, we havewhich nicely rearranges into. Hence, AB is 9 so our answer is .24、Tina randomly selects two distinct numbers from the setand Sergio randomly selects a number from the set. The probability that Sergio's number is larger than the sum of the two numbers chosen by Tina isSolutionThis is not too bad using casework.Tina gets a sum of 3: This happens in only one way (1,2) and Sergio can choose a number from 4 to 10, inclusive. There are 7 ways that Sergio gets a desirable number here.Tina gets a sum of 4: This once again happens in only one way (1,3). Sergio can choose a number from 5 to 10, so 6 ways here.Tina gets a sum of 5: This can happen in two ways (1,4) and (2,3). Sergio can choose a number from 6 to 10, so 2*5=10 ways here.Tina gets a sum of 6: Two ways here (1,5) and (2,4). Sergio can choose a number from 7 to 10, so 2*4=8 here.Tina gets a sum of 7: Two ways here (2,5) and (3,4). Sergio can choose from 8 to 10, so 2*3=6 ways here.Tina gets a sum of 8: Only one way possible (3,5). Sergio chooses 9 or 10, so 2 ways here.Tina gets a sum of 9: Only one way (4,5). Sergio must choose 10, so 1 way.In all, there are ways. Tina chooses twodistinct numbers in ways while Sergio chooses a number inways, so there are ways in all. Since , ouranswer is .25、In trapezoid with bases and , we have ,, , and . The area of isSolutionSolution 1It shouldn't be hard to use trigonometry to bash this and find the height, but there is a much easier way. Extend and to meet atpoint :Since we have , with the ratio ofproportionality being . Thus So the sides of are , which we recognize to be aright triangle. Therefore (we could simplify some of the calculation using that the ratio of areas is equal to the ratio of the sides squared),Solution 2Draw altitudes from points and :Translate the triangle so that coincides with . We getthe following triangle:The length of in this triangle is equal to the length of the original, minus the length of . Thus .Therefore is a well-known right triangle. Its area is, and therefore its altitude is.Now the area of the original trapezoid is.。

2021年美国数学竞赛AMC12A 真题加答案解析

2021 AMC 12A Peeyush Pandaya et al.February 20211 Answer1.B2. D3. D4.D5.E Key6.C7.D8.C9.C10.E11.C12.A13.B14.E15. D16.C17.D18.E19.C20.B21.A22.D23.D24.D25.E2 Problems and SolutionsProblem 1. What is the value of21+2+3- (2¹+2²+23)?(A)0 (B)50 (C)52 (D)54 (E)57Solution.2⁶-(2¹+2²+23)=64-(2+4+8)=64-14=(B)50Problem 2.Under what conditionsisva²+62=a+btrue,whereaand bare real numbers?(A)It is never true(B) It is true if and only ifab=0(C) It is true if and only ifa+b≥0(D)It is true ifandonlyifab=0anda+b≥0(E)It is always trueSolution. It is clear that both sides of the equation must be nonnegative.Squaring,a²+b²=a²+2ab+b2→ab=0The answer is (D)Problem 3.The sum of two natural numbers is 17,402.One of the two numbers is divisible by 10.If the units digit of that number is erased, the other number is obtained. What is the difference of these two numbers?(A)10,272 (B)11,700 (C)13,362 (D)14,238 (E)15,426Solution. Let the first number mentioned be 10n;the second is n. Then10n+n=17,402,from which it follows thatProblem 4. Tom has a collection of 13 snakes,4 of which are purple and 5 of which are happy. He observes that●all of his happy snakes can add,·none of his purple snakes can subtract●all of his snakes that can't subtract also can't add.Which of these conclusions can be drawn about Tom's snakes?Solution. Together, the second and third conditions imply that none of Tom's purple snakes can add.Thus,(D) is correct: happy snakes are not purple.Problem 5.When a student multiplied the number 66 by the repeating decimal,where a and b are digits, he did not notice the notation and just multiplied 66 times 1.ab. Later he found that his answer is 0.5 less than the correct answer. What is the 2-digit integer gb? (A)15 (B)30 (C)45 (D)60 (E)75Solution. The student computed 66 ; the correct answer is 66 . Thus,Problem 6.A deck of cards has only red cards and black cards. The probability of a randomly chosen card being red is . When 4 black cards are added to the deck, the probability of choosing red becomes . How many cards were in the deck originally?(A)6 (B)9 (C) 12 (D)15 (E)18Solution. If the deck begins with x red cards and 3x cards in total, thenProblem 7.What is the least possible value of(ry- 1)²+(x+y)2 for real numbersa and y?Solution. We have(ry- 1)2+(x+y)2=(ry)2-2ry+1+x²+2ry+v²=x2v²+z²+y2+1= (r²+1)(y²+1),which achieves a minimum of (D)1 atx=y=0.D Do D ₁ D ₂D ₃ D ₁ D ₅D ₆ D ₇ D ₈ D, D1o Problem 8.A sequence of numbers isdefinedbyDo=0,D ₁=0,Dz=1,andDn=Dn- 1+Dn-3 forn≥3.What are the parities(evenness oroddness)of the triple of numbers(D2021,D2022,D2023), whereE denotes even and O denotes odd?Solution.0/10 01 D2+Do=1+0=1 D ₃+Di=1+0=1D ₄+Dz=1+1=0 Ds+D ₃=0+1=1D ₆+D4=1+1=0D-+Ds=0+0=0D ₈+D ₆=0+1=1Dg+D ₇=1+0=1We can see that the pattern repeats in cycles of length7.and as 2021=5 mod7,we have D2021= Ds,D2022=D6,D2023=D7→(C)(E,O,E) Problem 9.Which of the following is equivalent to(2+3)(2²+3²)(2⁴+34)(2⁸+3⁸)(216+316)(2³²+3³2)(264+364)?(A)3127+2127 (B)3127+2127+2.363+3.263 (C)3128-2128 (D)3128+2128 (E)5127Solution.(3-2)(2+3)(2²+3²)(2⁴+3⁴)(2⁸+3⁸)(216+316)(2³²+3³2)(264+364)=(3²-2²)(2²+3²)(2⁴+3⁴)(2⁸+3⁸)(216+316)(232+3³2)(264+364)=(3⁴-2⁴)(2⁸+3⁸)(216+316)(232+332)(264+364)=(C)3128-2129=(364-264)(264+364)Problem 10.Two right circular cones with vertices facing down as shown in the figure below contain the same amount of liquid. The radii of the tops of the liquid surfaces are 3cm and 6cm. Into each cone is dropped a spherical marble of radius lcm, which sinks to the bottom and is completely submerged without spilling any liquid. What is the ratio of the rise of the liquid level the narrow cone to the rise of the liquid level in the wide cone?(A)1:1 (B)47:43 (C) 2:1 (D)40:13 (E)4:1Solution. The two cones have equal volume, so the height of the first is times that of the second. Since the volumes increase by equal proportions, the heights increase by equal proportions. Thus, the ratio of the rise in liquid levels is (E)4:1Problem 11.A laser is placed at the point(3,5). The laser beam travels in a straight rry wants the beam to hit and bounce off the y-axis, then hit and bounce off the x-axis,then hit the point (7,5). What is the total distance the beam will travel along this path?(A)2√10 (B)5√2(C)10√2(D)15√2 (E)10√5Solution. Reflect about the y-axis then the z-axis. It is well-known that the image under the two reflections must be a straight line.The answer isv(3-(-7))²+(5-(-5)= (C) 10√2Problem 12.All the roots of polynomialz6- 10z ⁵+Az ⁴+Bz³+Cz²+Dz+16are positiveintegers, possibly repeated. What is the value of B?(A)-88 (B)-80 (C)-64 (D)-41 (E)-40Solution. By Vieta's,the sum of the 6 roots is 10 and the product is 16,hence they are all powers of 2. It is not hard to find that the only working unordered sextuple is(2,2,2,2,1,1). As(z-2)4=24-8z3+24z2-32z+16 and(z- 1)2=z2-2z+1.the z3 coefficient is -8.1+24 · (-2)+(-32) · 1= (B)-88Problem 13.Of the following complex numbers z, which has the property that z5 has the greatest real part?(A)-2 (B)-√3+i (C)-√2+√2i Solution. The magnitude of each complex number is the same, so it suffices to look at the argu- ment. The angles are π, ,and ,which after raising to the 5th power give π, and . We seek the angle that reaches farthest to the right(smallest argument),which is . Thus, our answer is (B)-√3+iProblem 14.What is the value of(A)21 (B)100logs3 (C)200log35 (D)2,200 (E)21,000Solution.And,Therefore, their product is210logs3.100log35=(E)21,000(D)- 1+√3i (E)2iProblem 15.A choir director must select a group of singers from among his 6 tenors and 8 basses. The only requirements are that the difference between the numbers of tenors and basses must be a multiple of 4,and the group must have at least one singer.Let be the number of groups that could be selected. What is the remainder when N is divided by 100?(A)47 (B)48 (C)83 (D)95 (E)96Solution. Suppose we mark down(1) the tenors that are in the group,and(2)the basses that aren't in the group. Then we necessarily mark down a number of people that is a multiple of 4. This is also sufficient; we mark down some people numbering a multiple of 4, then select the marked tenors and unmarked basses to form our choir. Clearly, we just mark down at least one person. The answer is thus(D)95Problem 16.In the following list of numbers, the integer n appears times in the list for l≤n≤200.1,2,2,3,3,3,4,4,4,4,.…,200,200,...200.What is the median of the numbers in this list?(A)100.5 (B)134 (C)142 (D)150.5 (E)167Solution.For general n, we have numbers. We want to approximate a such that is close to . Since the formula is a quadratic in n and we are halving this value,we can find that a is approximately .Plugging in n = 200,this is about 100√2,or 141.Of the answer choices, (C)142 is the closest, and indeed it is our answer.To verify, we can see that and ), so clearly 142 works.Problem 17.Trapezoid ABCD has ABICD,BC=CD=43,and AD1BD.Let O be the intersection of the diagonals AC and BD,and let P be the midpoint of BD.Given that OP=11, the length AD can be written in the form myn,where m and n are positive integers and n is not divisible by the square of any prime. What is m+n?(A)65 (B)132 (C)157 (D)194 (E)215Solution. Let M be the intersection of CPand AB.Since DCBMisakite,andCMIBD,we have MP1PB,and by considering the homothethy taking △MBD to △ABD with scale factor 2,we can see that M is the midpoint of AB.In particular,we haveSince AD 1BD,we have AD1DOandthusZADO=90°,andasCD=CB,wehaveCP1BD and ZCPD=2CPO=90°.Also,ZAOD=ZCOP,so △AOD~ △COP.Therefore,so DO=22.Thus,AD=√AB²-BD²=√86²-66²=4√ 190→m+n= (D)194The desired answer isProblem 18.Let f be afunction defined on the set of positive rational numbers with the property that f(a ·b)=f(a)+f(b)for all positive rational numbersa and b.Suppose that falso has the property that f(p)= pfor every prime numberp.For which of the following numbers zis f(x)<0?(A)整(B) (C) (D) (E) 51Solution. Note that f(a ·1)=f(a)+f(1)= f(1)=0,andIn particular, it follows by induction that f(p*)= kp for each k ∈Z.Thus,(A) f(2-5. 17)=-5·2+17=7(B) f(2-4. 11)=-4·2+11=3(C) f(3-2.7)=-2.3+7=1(D)f(2- 1.3- 1.7)=- 1.2+(- 1) ·3+7=2(E)f(52.11- 1)=2.5- 11=- 125The answer is (E)11Problem 19.How many solutions does the equation sinclosed interval [0,π]?(A)0 (B)1 (C) 2 (D) 3 (E)4Solution. Note on the interval , the left-hand side is negative while the right-hand side is positive.We thus restrict our attention to ]. The arguments cosx and sz are both between 0 and .ForsinA=cosBinA,B ∈[0,],we must have . This implies sinz+cosz=1,hence . There are (C) 2 solutions.Problem 20.Suppose that on a parabola with vertexV and focus F there exists a point Asuch that AF=20 and AV=21.What is the sum of all possible values of the length FV?(A)13 (B) 40 (C) (D)14 (E)Solution. Let the directrix be the x-axis,F=(0,2d),V=(0,d),A=(x,y),andB=(0,y)for some d>0.By the definition of a parabola,y=20.We compute x in two ways:x²=AF²-BF²=20²-|20-2d|2=AV²-BV²=21²-|20-d²Subtracting,O=20²-21²+(20-d)²-(20-2d)2=3d²-40d+41.The sum of all possible values ofProblem 21.The five solutions to the equation(z- 1)(z²+2z+4)(z²+4z+6)=0may be written in theformzk+ykiforl≤k≤5,where xk and yk are real.Let E be the unique ellipse that passes through the points(Ti,yi),(x2,32),(r3,Y3),(x4,y4)and(xs,ys).The eccentricity ofE can be written in the form ,where m and n are relatively prime positive integers. What is m+n?(Recall that the eccentrictiy of an ellipse E is the ratio,where 2a is the length of the major axis ofE and 2c is the distance between its two foci.)(A)7 (B)9 (C)11 (D)13 (E)15Solution. The roots of the polynomial arez=1,z=- 1±i√3,andz=-2±i√2,hence the five points onE are(1,0),( - 1,土√3),( - 2,±√2) .By symmetry through the x-axis, the ellipse is of the formE: a(x-r)²+by²=1.We then have the relationsa(1-r)²=1a(1+r)²+3b=1a(2+r)²+2b=1.Eliminating b from the latter two,1=3[a(2+r)²+2]-2[a(1+r)²+36]=a(r²+8r+10),henceit follows that and so the eccentricity is 1/√6. The requested sum is1+6= (A)7Problem 22.Suppose that the roots of the polynomialP(x)=x³+ax²+bx+care cos 2π,COS 47 and cos ,where angles are in radians. What is abc?(C) (D) (E)Solution. Recall1+e2m/7+ …+e12mi/7=0→e2mi/7+e4xi/7+e6mi/7=- 1/2,and in particular caNote are solutions to the equation cosb+cos28+cos30=- 1/2,so lettingx=co sθimplies thathas roots ,COS 4π7Thus, the polynomial in question is and the requested answer isRemark. Perhaps it is easier to motivate the solution as follows.Lett=e2mi/7andx=t+t- 1= 2cos2π/7.Thenx²-2=t²+t-2andx³-3x=t³+t-3.Moreover,t⁶+t⁵+ …+1=0impliest³+t²+t+t- 1+t-2+t-3=- 1,i.e.x+(x²-2)+(r³-3r) has root 2cos2π/7.It certainly seems logical that the Galois conjugates of 2cos would be 2cos and 2cos (especially given the phrasing of the problem),so simply replacex → to get the desired polynomial form.Let w = e2ix/7.Note thatLet these be r,s,t respectively. By Vieta's formulas, note that the desired quantity is(-rst)(rs+st+tr)(-r-s-t)=(r+s+t)(rs+st+tr)(rst).Note that 1+w+ …+w⁶=0.We haveThen,FinallyMultiplying yields the answer of (D)1 32Solution Manual 2021AMC12AProblem 23.Frieda the frog begins a sequence of hops on a 3×3 grid of squares,moving one square on each hop and choosing at random the direction of each hop up,down, left,or right. She does not hop diagonally. When the direction of a hop would take Frieda off the grid,she ”wraps around”and jumps to the opposite edge.For example if Frieda begins in the center square and makes two hops ”up”,the first hop would place her in the top row middle square, and the second hop would cause Frieda to jump to the opposite edge,landing in the bottom row middle square.Suppose Frieda starts from the center square, makes at most four hops at random,and stops hopping if she lands on a corner square. What is the probability that she reaches a corner square on one of the four hops?(A)G (B) (D)强(E)8Solution. We complementary count,and determine the probability we never reach a corner square. Denote by A the center,and B a square adjacent to the center. Then the first hop lands on B.● If the second hop lands on A(with probability ,then the third hop lands on B always,andthere is a chance the fourth hop lands on a non-corner square. The probability in this case is● If the second hop lands on B, then there is a chance the third hop lands on A,and achance the third hop lands on B.In the former subcase, the fourth hop always lands on a non-corner square, and in the latter subcase, there is a chance the fourth hop lands on a non-corner square. The probability in this case isThe requested probability isProblem 24.SemicircleT has diameter AB of length14.Circle Ωlies tangent to AB at a point P and intersectsI at pointsQandR.IfQR=3√3and ZQPR=60°,then the area of △PQR is ,where a and c are relatively prime positive integers, and b is a positive integer not divisible by the square of any prime. What isa+b+c?(A)110 (B)114 (C)118 (D)122 (E)126Solution. First, by Extended Law of Sines,we have that the radius of Q is .Let M be the midpoint of QR,O be the center ofT,and X be the center of 2.Since △OQR is isosceles, OM is perpendicular to QR. Thus we have that X lies on OM since it is the circumcenter of △puting lengths, we hav thagorean theorem on △ORM andfrom isosceles triangle XQR. Thus and OP=4 from Pythagorean theorem on △OXP.To find [PQR],we will find the height from P to QR.Let the foot of the perpendicular fromX to the P-altitude be D. Since PDⅡOM,we know that △XDP~△OPX.This means that . Now note that the bottom portion of the P-altitude after subtractingPD is equal to XM,so the height of the triangle is . The area is simply2021 AMC 12A11 Solution ManualProblem 25.Let d(n)denote the number of positive integers that divide n,including l and n. For example,d(1)=1,d(2)=2,and d(12)=6.(This function is known as the divisor function.) LetThere is a unique positive integer N such that f(N)> f(n)for all positive integers n≠N.What is the sum of the digits of N?(A)5 (B)6 (C)7 (D)8 (E) 9Solution. Letn=II;p',wherepi=2,Pz=3,etc.are the primes in increasing order and e; are nonnegative integers. ThenIt is equivalent to maximize Thus, it remains to find the optimal e; for each i. We go term-by-term, noting that it is only necessary to check until the expression first decreases, as exponentials increase more quickly than polynomials.ei 0 1 2 3 4 0 1 2 3 0 1 2 0 1 2 ((ez+1)3)/p⁸1³/20=1 23/2¹=433/2²=6.25 4³/2³=85³/2⁴<8 13/3⁰=1 23/31≈2.67 3³/32=3 43/3³<3 13/5⁰=1 2³/5¹=1.6 3³/5²=1.08 13/70=1 23/71≈1.14 3³/7²<1Note that we do not need to check p≥11,ase;=1yields which is suboptimal.Thus,the answer isN=23.32.5.7=2520,which has a Remark:It is sufficient to stop at p=3,for 3²|Nleaves N among the answer choices.digit sum of (E)9only one possibility for the digit sum ofi 1 1 1 1 1 2 2 2 2 3 3 3 4 4 4。

美国数学竞赛AMC8 -- 2006年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 – 2006年真题解析(英文解析+中文解析) \Problem 1Answer: DSolution:The three prices round to $2, $5, and $10, which has a sum of 17.中文解析：三件商品价格先近似取整，然后求和：2+5+10=17. 答案是D。

Problem 2Answer: CSolution:As the AMC 8 only rewards 1 point for each correct answer, everything is irrelevant except the number Billy answered correctly，13.中文解析：正确的题目每题1分，错误或没做的题目都是0分，做对13题的得分应该是13. 答案是C。

Problem 3Answer: ASolution:When Elisa started, she finished a lap in 25/10=2.5 minutes. Now, she finishes a lap is 24/12=2 minutes. The difference is 2.5-2=0.5中文解析：开始25分钟游10圈，平均2.5分钟游1圈。

后来24分钟游12圈，平均2分钟游1圈。

速度从2.5分钟提高到2分钟，提高了0.5分钟，即1/2 分钟。

答案是A。

Problem 4Answer: BSolution:If the spinner goes clockwise 2+1/4 revolutions and then counterclockwise 3+3/4 revolutions, it ultimately goes counterclockwise 1+1/2 which brings the spinner pointing east.中文解析：最初方向指向西，转整数圈不改变指针方向。

2020 AMC 10B (美国数学竞赛)真题加详解

2020 AMC 10B Solution Problem1What is the value ofSolutionWe know that when we subtract negative numbers, .The equation becomesProblem2Carl has cubes each having side length , and Kate has cubes each having side length . What is the total volume of these cubes?SolutionA cube with side length has volume , so of these will have a total volume of .A cube with side length has volume , so of these will have a total volume of .~quacker88Problem 3The ratio of to is , the ratio of to is , and the ratioof to is . What is the ratio of toSolution 1WLOG, let and .Since the ratio of to is , we can substitute in the value of toget .The ratio of to is , so .The ratio of to is then so our answeris ~quacker88Solution 2We need to somehow link all three of the ratios together. We can start by connecting the last two ratios together by multiplying the last ratio by two., and since , we can link themtogether to get .Finally, since , we can link this again to get: ,so ~quacker88Problem4The acute angles of a right triangle are and , where andboth and are prime numbers. What is the least possible value of ?SolutionSince the three angles of a triangle add up to and one of the anglesis because it's a right triangle, .The greatest prime number less than is . If ,then , which is not prime.The next greatest prime number less than is . If ,then , which IS prime, so we have our answer ~quacker88 Solution 2Looking at the answer choices, only and are coprime to . Testing , the smaller angle, makes the other angle which is prime, therefore our answerisProblem5How many distinguishable arrangements are there of 1 brown tile, 1 purple tile, 2 green tiles, and 3 yellow tiles in a row from left to right? (Tiles of the same color are indistinguishable.)SolutionLet's first find how many possibilities there would be if they were all distinguishable, then divide out the ones we overcounted.There are ways to order objects. However, since there's ways to switch the yellow tiles around without changing anything (since they're indistinguishable) and ways to order the green tiles, we have to divide out these possibilities.~quacker88SolutionWe can repeat chooses extensively to find the answer. Thereare choose ways to arrange the brown tiles which is . Then from the remaining tiles there are choose ways to arrange the red tiles. And now from the remaining two tiles and two slots we can see there are two ways to arrange the purple and brown tiles, giving us an answerofProblem6Driving along a highway, Megan noticed that her odometershowed (miles). This number is a palindrome-it reads the same forward and backward. Then hours later, the odometer displayed the next higher palindrome. What was her average speed, in miles per hour, during this -hour period?SolutionIn order to get the smallest palindrome greater than , we need to raise the middle digit. If we were to raise any of the digits after the middle, we would be forced to also raise a digit before the middle to keep it a palindrome, making it unnecessarily larger.So we raise to the next largest value, , but obviously, that's not how place value works, so we're in the s now. To keep this a palindrome, our number is now .So Megan drove miles. Since this happened over hours, she drove at mph. ~quacker88 Problem7How many positive even multiples of less than are perfect squares?SolutionAny even multiple of is a multiple of , so we need to find multiples of that are perfect squares and less than . Any solution that we want will be in theform , where is a positive integer. The smallest possible value isat , and the largest is at (where the expression equals ). Therefore, there are a total of possible numbers.-PCChess Problem8Points and lie in a plane with . How many locations forpoint in this plane are there such that the triangle with vertices , ,and is a right triangle with area square units?Solution 1There are options here:1. is the right angle.It's clear that there are points that fit this, one that's directly to the rightof and one that's directly to the left. We don't need to find the length, we just need to know that it is possible, which it is.2. is the right angle.Using the exact same reasoning, there are also solutions for this one.3. The new point is the right angle.(Diagram temporarily removed due to asymptote error)The diagram looks something like this. We know that the altitude tobase must be since the area is . From here, we must see if there are valid triangles that satisfy the necessary requirements.First of all, because of the area.Next, from the Pythagorean Theorem.From here, we must look to see if there are valid solutions. There are multiple ways to do this:We know that the minimum value of iswhen . In this case, the equationbecomes , which is LESSthan . . The equationbecomes , which is obviously greater than . We canconclude that there are values for and in between that satisfy the Pythagorean Theorem.And since , the triangle is not isoceles, meaning we could reflectit over and/or the line perpendicular to for a total of triangles this case.Solution 2Note that line segment can either be the shorter leg, longer leg or thehypotenuse. If it is the shorter leg, there are two possible points for that cansatisfy the requirements - that being above or below . As such, thereare ways for this case. Similarly, one can find that there are also ways for point to lie if is the longer leg. If it is a hypotenuse, then thereare possible points because the arrangement of the shorter and longer legs can be switched, and can be either above or below the line segment. Therefore, the answer is .Problem9How many ordered pairs of integers satisfy theequationSolutionRearranging the terms and and completing the square for yields theresult . Then, notice that can onlybe , and because any value of that is greater than 1 will causethe term to be less than , which is impossible as must be real. Therefore, plugging in the above values for gives the orderedpairs , , , and gives a totalof ordered pairs.Solution 2Bringing all of the terms to the LHS, we see a quadraticequation in terms of . Applying the quadratic formula, weget In order for to be real, which it must be given the stipulation that we are seekingintegral answers, we know that the discriminant, must benonnegative. Therefore, Here, we see that we must split the inequality into a compound, resultingin .The only integers that satisfy this are . Plugging thesevalues back into the quadratic equation, we see that both produce a discriminant of , meaning that there is only 1 solution for .If , then the discriminant is nonzero, therefore resulting in two solutions for .Thus, the answer is .~TiblisSolution 3, x firstSet it up as a quadratic in terms of y:Then the discriminant is This will clearly only yield real solutionswhen , because it is always positive. Then . Checking each one: and are the same when raised to the 2020th power:This has only has solutions , so are solutions. Next, if :Which has 2 solutions, so andThese are the only 4 solutions, soSolution 4, y firstMove the term to the other side toget . Because for all , then . If or , the right side is and therefore . When , the right side become , therefore . Our solutions are , , , . There are solutions, so the answer is - wwt7535Problem 10A three-quarter sector of a circle of radius inches together with its interior can be rolled up to form the lateral surface area of a right circular cone by taping together along the two radii shown. What is the volume of the cone in cubicinches?SolutionNotice that when the cone is created, the radius of the circle will become the slant height of the cone and the intact circumference of the circle will become the circumference of the base of the cone.We can calculate that the intact circumference of the circle is . Since that is also equal to the circumference of the cone, the radius of the cone is . We also have that the slant height of the cone is . Therefore, we use the Pythagorean Theorem to calculate that the height of the coneis . The volume of the coneis -PCChessSolution 2 (Last Resort/Cheap)Using a ruler, measure a circle of radius 4 and cut out the circle and then the quarter missing. Then, fold it into a cone and measure the diameter to be 6cm . You can form a right triangle with sides 3, 4, and then through the Pythagorean theorem the height is found tobe . The volume of a cone is . Plugging in we findProblem11Ms. Carr asks her students to read any 5 of the 10 books on a reading list. Harold randomly selects 5 books from this list, and Betty does the same. What is the probability that there are exactly 2 books that they both select?SolutionWe don't care about which books Harold selects. We just care that Bettypicks books from Harold's list and that aren't on Harold's list.The total amount of combinations of books that Betty can selectis .There are ways for Betty to choose of the books that are on Harold's list.From the remaining books that aren't on Harold's list, thereare ways to choose of them.~quacker88Problem12The decimal representation of consists of a string of zeros after the decimal point, followed by a and then several more digits. How many zeros are in that initial string of zeros after the decimal point?Solution 1Now we do some estimation. Notice that , which meansthat is a little more than . Multiplying itwith , we get that the denominator is about . Notice that whenwe divide by an digit number, there are zeros before the first nonzero digit. This means that when we divide by the digitinteger , there are zeros in the initial string after the decimal point. -PCChessSolution 2First rewrite as . Then, we know that when we write this in decimal form, there will be 40 digits after the decimal point. Therefore, we just have to findthe number of digits in .and memming (alternatively use the factthat ),digits.Our answer is .Solution 3 (Brute Force)Just as in Solution we rewrite as We thencalculate entirely by hand, first doing then multiplying that product by itself, resulting in Because this is digits,after dividing this number by fourteen times, the decimal point is beforethe Dividing the number again by twenty-six more times allows a stringof zeroes to be formed. -OreoChocolateSolution 4 (Smarter Brute Force)Just as in Solutions and we rewrite as We can then look at the number of digits in powersof . , , , , ,, and so on. We notice after a few iterations that every power of five with an exponent of , the number of digits doesn't increase. This means should have digits since thereare numbers which are from to , or digits total. This means our expression can be written as , where is in therange . Canceling gives , or zeroes before the since the number should start on where the one would be in . ~aop2014 Solution 5 (Logarithms)Problem13Andy the Ant lives on a coordinate plane and is currently at facingeast (that is, in the positive -direction). Andy moves unit and thenturns degrees left. From there, Andy moves units (north) and thenturns degrees left. He then moves units (west) and againturns degrees left. Andy continues his progress, increasing his distance each time by unit and always turning left. What is the location of the point at which Andy makes the th leftturn?Solution 1You can find that every four moves both coordinates decrease by 2. Therefore, both coordinates need to decrease by two 505 times. You subtract, giving you theanswer of ~happykeeperProblem14As shown in the figure below, six semicircles lie in the interior of a regular hexagon with side length 2 so that the diameters of the semicircles coincide with the sides of the hexagon. What is the area of the shaded region — inside the hexagon but outside all of the semicircles?Solution 1Let point A be a vertex of the regular hexagon, let point B be the midpoint of the line connecting point A and a neighboring vertex, and let point C be the second intersection of the two semicircles that pass through point A. Then, , since B is the center of the semicircle with radius 1 that C lies on, , since B is the center of the semicircle with radius 1 that A lies on,and , as a regular hexagon has angles of 120,and is half of any angle in this hexagon. Now, using the sinelaw, , so . Since the angles in a triangle sum to 180, is also 60. Therefore, is an equilateral triangle with side lengths of 1.Since the area of a regular hexagon can be found with the formula , where is the side length of the hexagon, the area of this hexagonis . Since the area of an equilateral triangle can be foundwith the formula , where is the side length of the equilateral triangle,the area of an equilateral triangle with side lengths of 1 is . Since the area of a circle can be found with the formula , the area of a sixthof a circle with radius 1 is . In each sixth of the hexagon, thereare two equilateral triangles colored white, each with an area of , and onesixth of a circle with radius 1 colored white, with an area of . The rest of the sixth is colored gray. Therefore, the total area that is colored white in each sixthof the hexagon is , which equals , and the total areacolored white is , which equals . Since the area colored gray equals the total area of the hexagon minus the area colored white,the area colored gray is , whichequals .Solution 2First, subdivide the hexagon into 24 equilateral triangles with side length1:Now note that the entire shadedregion is just 6 times this part:The entire rhombus is just 2 equilatrial triangles with side lengths of 1, so it has an area of:The arc that is not included has an area of:Hence, the area ofthe shaded region in that section is For a final areaof:Problem15Steve wrote the digits , , , , and in order repeatedly from left to right, forming a list of digits, beginning He thenerased every third digit from his list (that is, the rd, th, th, digits from the left), then erased every fourth digit from the resulting list (that is, the th, th, th, digits from the left in what remained), and then erased every fifth digit from what remained at that point. What is the sum of the three digits that were then in the positions ?Solution 1After erasing every third digit, the list becomes repeated. After erasing every fourth digit from this list, the listbecomes repeated. Finally, after erasing every fifth digit from this list, the list becomes repeated. Since this list repeats every digits andsince are respectively in we have that the th, th, and st digits are the rd, th, and thdigits respectively. It follows that the answer is~dolphin7Problem16Bela and Jenn play the following game on the closed interval of the real number line, where is a fixed integer greater than . They take turns playing, with Bela going first. At his first turn, Bela chooses any real number in theinterval . Thereafter, the player whose turn it is chooses a real numberthat is more than one unit away from all numbers previously chosen by either player. A player unable to choose such a number loses. Using optimal strategy, which player will win the game?SolutionNotice that to use the optimal strategy to win the game, Bela must select themiddle number in the range and then mirror whatever number Jennselects. Therefore, if Jenn can select a number within the range, so can Bela. Jenn will always be the first person to run out of a number to choose, so theanswer is .Solution 2 (Guessing)First of all, realize that the value of should have no effect on the strategy at all. This is because they can choose real numbers, not integers, so even if is odd, for example, they can still go halfway. Similarly, there is no reason the strategy would change when .So we are left with (A) and (B). From here it is best to try out random numbers and try to find the strategy that will let Bela win, but if you can't find it, realize thatit is more likely the answer is since Bela has the first move and thus has more control.Problem17There are people standing equally spaced around a circle. Each person knows exactly of the other people: the people standing next to her or him, as well as the person directly across the circle. How many ways are there forthe people to split up into pairs so that the members of each pair know each other?SolutionLet us use casework on the number of diagonals.Case 1: diagonals There are ways: either pairs with , pairs with , and so on or pairs with , pairs with , etc.Case 2: diagonal There are possible diagonals to draw (everyone else pairs with the person next to them.Note that there cannot be 2 diagonals.Case 3: diagonalsNote that there cannot be a case with 4 diagonals because then there would have to be 5 diagonals for the two remaining people, thus a contradiction.Case 4: diagonals There is way to do this.Thus, in total there are possible ways. Problem18An urn contains one red ball and one blue ball. A box of extra red and blue balls lie nearby. George performs the following operation four times: he draws a ball from the urn at random and then takes a ball of the same color from the box and returns those two matching balls to the urn. After the four iterations the urn contains six balls. What is the probability that the urn contains three balls of each color?SolutionLet denote that George selects a red ball and that he selects a blue one. Now, in order to get balls of each color, he needs more of both and .There are 6cases:(wecan confirm that there are only since ). However we canclump , ,and together since they are equivalent by symmetry.andLet's find the probability that he picks the balls in the order of .The probability that the first ball he picks is red is .Now there are reds and blue in the urn. The probability that he picks red again is now .There are reds and blue now. The probability that he picks a blue is .Finally, there are reds and blues. The probability that he picks a blue is . So the probability that the case happensis . However, since the case is the exactsame by symmetry, case 1 has a probability of chance of happening.andLet's find the probability that he picks the balls in the order of .The probability that the first ball he picks is red is .Now there are reds and blue in the urn. The probability that he picks blue is .There are reds and blues now. The probability that he picks a red is . Finally, there are reds and blues. The probability that he picks a blue is .So the probability that the case happensis . However, since the case is the exactsame by symmetry, case 2 has a probability of chance of happening.andLet's find the probability that he picks the balls in the order of .The probability that the first ball he picks is red is .Now there are reds and blue in the urn. The probability that he picks blueis .There are reds and blues now. The probability that he picks a blue is .Finally, there are reds and blues. The probability that he picks a red is .So the probability that the case happensis . However, since the case is the exactsame by symmetry, case 3 has a probability of chance of happening.Adding up the cases, we have ~quacker88 Solution 2We know that we need to find the probability of adding 2 red and 2 blue balls insome order. There are 6 ways to do this, since there are ways to arrange in some order. We will show that the probability for each of these 6 ways is the same.We first note that the denominators should be counted by the same number. This number is . This is because 2, 3, 4, and 5 represent how many choices there are for the four steps. No matter what the stepinvolves numbers to choose from.The numerators are the number of successful operations. No matter the order, the first time a red is added will come from 1 choice and the second time will come from 2 choices, since that is how many reds are in the urn originally. Thesame goes for the blue ones. The numerator must equal . Therefore, the probability for each of the orderingsof is . There are 6 of these, so the total probabilityis .Solution 3First, notice that when George chooses a ball he just adds another ball of the same color. On George's first move, he either chooses the red or the blue witha chance each. We can assume he chooses Red(chance ), and then multiply the final answer by two for symmetry. Now, there are two red balls andone blue ball in the urn. Then, he can either choose another Red(chance ), in which case he must choose two blues to get three of each, withprobability or a blue for two blue and two red in the urn, withchance . If he chooses blue next, he can either choose a red then a blue, or ablue then a red. Each of these has a for total of . The total probability that he ends up with three red and three blueis . ~aop2014 Solution 4Let the probability that the urn ends up with more red balls be denoted . Since this is equal to the probability there are more blue balls, the probabilitythere are equal amounts is . the probability no more blues are chosen plus the probability only 1 more blue is chosen. The firstcase, .The second case, . Thus,the answer is .~JHawk0224Solution 5By conditional probability after 4 rounds we have 5 cases: RRRBBB, RRRRBB,RRBBBB, RRRRRB and RBBBBB. Thus the probability is . Put .~FANYUCHEN20020715Edited by KinglogicSolution 6Here X stands for R or B, and Y for the remaining color. After 3 rounds one can either have a 4+1 configuration (XXXXY), or 3+2 configuration (XXXYY). Theprobability of getting to XXXYYY from XXXYY is . Observe that the probability of arriving to 4+1 configuration is ( to get from XXY toXXXY, to get from XXXY to XXXXY). Thus the probability of arriving to 3+2configuration is also , and the answer isSolution 7We can try to use dynamic programming to solve this problem. (Informatics Olympiad hahaha)We let be the probability that we end up with red balls and blue balls. Notice that there are only two ways that we can end up with red balls and blue balls: one is by fetching a red ball from the urn when wehave red balls and blue balls and the other is by fetching a blue ball from the urn when we have red balls and blue balls.Then wehaveThen we start can with and try to compute .The answer is .Problem19In a certain card game, a player is dealt a hand of cards from a deckof distinct cards. The number of distinct (unordered) hands that can be dealt to the player can be written as . What is the digit ?Solution 1We're looking for the amount of ways we can get cards from a deck of ,which is represented by .We need to get rid of the multiples of , which will subsequently get rid of the multiples of (if we didn't, the zeroes would mess with the equation since you can't divide by 0), , leaves us with 17.Converting these into, we have~quacker88 Solution 2Since this number is divisible by but not , the last digits must be divisible by but the last digits cannot be divisible by . This narrows the options down to and .Also, the number cannot be divisible by . Adding up the digits, weget . If , then the expression equals , a multiple of . This would mean that the entire number would be divisible by , which is not what we want. Therefore, the only option is -PCChessSolution 3It is not hard to check that divides thenumber,As , using wehave .Thus , implying so the answer is .Solution 4As mentioned above,We can divide both sidesof by 10 to obtain which means is simply the units digit of the left-hand side. This valueisProblem20Let be a right rectangular prism (box) with edges lengths and ,together with its interior. For real , let be the set of points in -dimensional space that lie within a distance of some point . The volumeof can be expressed as ,where and are positive real numbers. What isSolutionSplit into 4 regions:1. The rectangular prism itself2. The extensions of the faces of3. The quarter cylinders at each edge of4. The one-eighth spheres at each corner ofRegion 1: The volume of is 12, soRegion 2: The volume is equal to the surface area of times . The surfacearea can be computed to be ,so .Region 3: The volume of each quarter cylinder is equal to . The sum of all such cylinders must equal times the sum of the edge lengths. This can be computed as , so the sum of the volumes of the quarter cylinders is , soRegion 4: There is an eighth of a sphere of radius at each corner. Since there are 8 corners, these add up to one full sphere of radius . The volume of thissphere is , so .Using these values,Problem21In square , points and lie on and , respectively, so that Points and lie on and , respectively, and points and lie on so that and . See the figure below. Triangle , quadrilateral ,quadrilateral , and pentagon each has area Whatis ?SolutionSince the total area is , the side length of square is . We see that since triangle is a right isosceles triangle with area 1, we can determinesides and both to be . Now, considerextending and until they intersect. Let the point of intersection be .We note that is also a right isosceles triangle with side and find it's area to be . Now, we notice that is also a rightisosceles triangle and find it's area to be . This is also equalto or . Since we are looking for , we want two times this. That gives .~TLiuSolution 2Since this is a geometry problem involving sides, and we know that is , we can use our ruler and find the ratio between and . Measuring(on the booklet), we get that is about inches and isabout inches. Thus, we can then multiply the length of by the ratioof of which we then get We take the square of that andget and the closest answer to that is . ~Celloboy (Note that this is just a strategy I happened to use that worked. Do not press your luck with this strategy, for it was a lucky guess)Solution 3Draw the auxiliary line . Denote by the point it intersects with , and by the point it intersects with . Last, denote by the segment , and by the segment . We will find two equations for and , and then solve for .Since the overall area of is ,and . In addition, the areaof .The two equations for and are then:Lengthof :Area of CMIF: .Substituting the first into the second,yieldsSolving for gives ~DrBSolution 4Plot a point such that and are collinear and extend line topoint such that forms a square. Extend line to meetline and point is the intersection of the two. The area of this square is equivalent to . We see that the area of square is , meaning each side is of length 2. The area of the pentagon is .Length , thus . Triangle is isosceles, and the area of this triangleis . Adding these two areas, we get . --OGBooger Solution 5 (HARD Calculation)We can easily observe that the area of square is 4 and its side length is 2 since all four regions that build up the square has area 1. Extend and let the intersection with be . Connect , and let the intersectionof and be . Notice that since the area of triangle is 1and , ,therefore . Let ,。

2021年美国数学竞赛(AMC10A)的试题与解答

2021年美国数学竞赛(AMC10A)的试题与解答华南师范大学数学科学学院(510631)李湖南1.算式(22−2)−(32−3)+(42−4)的值是多少?(A)1(B)2(C)5(D)8(E)12解直接计算,原式=2−6+12=8,故(D)正确.2.Portia高中的学生人数是Lara高中的3倍,这两所高中总共有2600名学生.问Portia高中有多少名学生?(A)600(B)650(C)1950(D)2000(E)2050解依题意可知,Portia高中占总人数的34,即有2600×34=1950名学生,故(C)正确.3.两个自然数之和是17402.这两个数中的一个可以被10整除,如果去掉该数的个位数字则得到另外一个数.问这两个数的差是多少?(A)10272(B)11700(C)13362(D)14238(E)15426解设第一个数为x,则第二个数为x10,已知x+x10=17402,解得x=15820,于是两数之差为x−x10=910x=14238,故(D)正确.4.一辆小车冲下山坡,它第一秒移动了5英寸,并且速度不断加快.在每个连续的1秒时间间隔内,它都比前1秒多移动7英寸.小车用了30秒到达山脚.问它一共行进了多少英寸?(A)215(B)360(C)2992(D)3195(E)3242解小车每秒移动的距离是个等差数列,首项为5,公差为7,第30秒移动了208英寸,从而距离和为(5+208)×30÷2=3195英寸,故(D)正确.5.一个共有k>12名学生的班级进行测验的平均分为8分,其中12名学生的测验平均分是14分.问其余学生的测验平均分如何用k来表示?(A)14−8k−12(B)8k−168k−12(C)1412−8k(D)14(k−12)k2(E)14(k−12)8k解所有学生的分数总和为8k,其中12名学生的分数和为168,剩下k−12名学生的分数和为8k−168,故平均分为8k−168k−12,(B)正确.6.Chantal和Jean从山路的起点开始向消防塔徒步旅行.Jean背着一个沉重的背包,走得较慢.Chantal开始以每小时4英里的速度行走,走到路程一半,山路变得非常陡峭,Chantal的速度减慢到每小时2英里.到达塔后,她立即掉头,以每小时3英里的速度沿着陡峭的山路向下走,她在路程的一半处遇见了Jean.从出发到他们相遇,Jean的平均速度是每小时多少英里?(A)1213(B)1(C)1312(D)2413(E)2解设半程为x英里,Jean所用的时间与Chantal一样,均为x4+x2+x3=13x12小时,于是Jean的平均速度为x(13x)/12=1213英里/小时,故(A)正确.7.汤姆有13条蛇,其中4条是紫色的,5条是快乐的.他观察发现:他的所有快乐的蛇都能做加法,他的紫色的蛇不会做减法,而且他所有不会做减法的蛇也不会做加法.关于汤姆的蛇,可以得出以下哪个结论?(A)紫色的蛇可以做加法(B)紫色的蛇是快乐的(C)能做加法的蛇是紫色的(D)快乐的蛇不是紫色的(E)快乐的蛇不能做减法解紫色的蛇不会做减法,从而也不会做加法,因此也不是快乐的.故快乐的蛇都不是紫色的,(D)正确.8.一名学生在用66乘以如下的循环小数时, 1.abab···=1.˙a˙b,其中a和b是数字.他没有注意到循环小数的标识,而只是做了66乘以1.ab.后来他发现他的答案比正确答案小0.5.问两位整数ab是多少?(A)15(B)30(C)45(D)60(E)75解由题意可得,0.5=66×(1.˙a˙b−1.ab)=66×0.00˙a˙b= 0.66×ab99,解得ab=75,故(E)正确.9.对于实数x和y,(xy−1)2+(x+y)2的最小可能值是多少?(A)0(B)14(C)12(D)1(E)2解(xy−1)2+(x+y)2=x2y2+x2+y2+1 1,此时x=y=0,故(D)正确.10.算式(2+3)(22+32)(24+34)(28+38)(216+ 316)(232+332)(264+364)与下面哪个表达式相等?(A)3127+2127(B)3127+2127+2×363+3×263(C)3128−2128(D)3128+2128(E)5127解连续使用平方差公式,可得原式=(3−2)(3+2)(32+22)(34+24)(38+28)(316+216)(332+232)(364+264)=3128−2128,故(C)正确.11.选择下面哪个整数b 为基数,可以使得b 进制数2021b −221b 不能被3整除?(A)3(B)4(C)6(D)7(E)8解由于2021b −221b =2×b 3−2×b 2=2b 2(b −1),当b =3,4,6,7时,该数均能被3整除,故(E)正确.12.如图所示,两个顶点朝下的正圆锥包含相同量的液体.液体顶部表面的半径分别为3厘米和6厘米.在每个圆锥体中放入一个半径为1厘米的球形弹子,它沉入底部,完全浸没,没有任何液体溢出.问窄圆锥内液面上升的高度与宽圆锥内液面上升的高度之比是多少?(A)1:1(B)47:43(C)2:1(D)40:13(E)4:1解设液体的体积为V ,左右两边液面高度分别为h 1,h 2,则V =13π32·h 1=13π62·h 2,因而h 1=4h 2.设放入弹子的体积为V 0=43π,左右两边液面上升高度分别为∆h 1,∆h 2,顶部液面的半径分别为r 1,r 2,则有V +V 0=13πr 21·(h 1+∆h 1)=13πr 22·(h 2+∆h 2),3r 1=h 1h 1+∆h 1,6r 2=h 2h 2+∆h 2,解得r 1r 2=12,∆h 1∆h 2=41,故(E)正确.13.四面体ABCD 中,各边长为AB =2,AC =3,AD =4,BC =√13,BD =2√5和CD =5,问它的体积是多少?(A)3(B)2√3(C)4(D)3√3(E)6解如图所示,由于AB 2+AC 2=BC 2,AB 2+AD 2=BD 2,AC 2+AD 2=CD 2,可得AB,AC,AD 互相垂直,即ABCD 是个直四面体.故V ABCD =13S ∆ABD ·AC =13·12×2×4·3=4,(C)正确.14.多项式z 6−10z 5+Az 4+Bz 3+Cz 2+Dz +16的根都是正整数,有可能重复.问B 的取值是多少?(A)−88(B)−80(C)−64(D)−41(E)−40解设多项式的根为x 1,x 2,x 3,x 4,x 5,x 6,其中均为正整数,则z 6−10z 5+Az 4+Bz 3+Cz 2+Dz +16=(z −x 1)(z −x 2)···(z −x 6),可得 x 1+x 2+x 3+x 4+x 5+x 6=10,x 1x 2x 3x 4x 5x 6=16,−∑i<j<kx i x j x k =B,解得x 1=x 2=1,x 3=x 4=x 5=x 6=2,于是B =−(C 14·12·2+C 12·C 24·1·22+C 34·23)=−88,故(A)正确.15.A,B,C 和D 的值从{1,2,3,4,5,6}中不重复地选取(即没有两个字母的取值相同),使得两条曲线y =Ax 2+B 和y =Cx 2+D 相交的不同取值方式有多少种?(不考虑曲线列出的顺序,例如,A =3,B =2,C =4,D =1与A =4,B =1,C =3,D =2被认为是相同的)(A)30(B)60(C)90(D)180(E)360解要使得y =Ax 2+B 和y =Cx 2+D 相交,则方程Ax 2+B =Cx 2+D 有解,即有(A −C )x 2=D −B ,此时A −C,D −B 的符号一样.任取A,C ∈{1,2,3,4,5,6},则D,B ∈{1,2,3,4,5,6}−{A,C },大小关系须一致,则有2C 26C 24=180种选择;另外不考虑曲线的顺序,故不同的取值方式共有180÷2=90种,(C)正确.16.在下面的数据列表中,对于1 n 200,整数n 出现了n 次.1,2,2,3,3,3,4,4,4,4,···,200,200,···,200.问这组数据列表中的中位数是多少?(A)100.5(B)134(C)142(D)150.5(E)167解这列数共有1+2+3+ (200)12×200×201=20100个,中位数是第10050与第10051个数的平均值.先求得12n (n +1) 10050的最大值为n max =141,且12n max (n max +1)=10011.这说明第10050和第10051个数均为142,故中位数为142,(C)正确.17.在梯形ABCD 中,AB //CD ,BC =CD =43,并且AD ⊥BD .设O 是对角线AC 和BD 的交点,P 是BD的中点.已知OP =11,AD 的长度可以表示成m √n ,其中m 和n 是正整数,并且n 不能被任何质数的平方所整除.问m +n 的值是多少?(A)65(B)132(C)157(D)194(E)215解如图所示,延长CP 交AB 于点E ,由于∆CBD 是等腰三角形,P 是BD 中点,从而CP⊥BD ,进而CE //DA ,于是AECD 是个平行四边形,得EA =CD =43.又∠CBD =∠CDB =∠ABD ,可得∆CBP =∆EBP ,即得BE =BC =43,于是AB =86.再根据∆CDO ∆ABO ,有OD OB =CD AB =12,即OD OB =BP −11BP +11=12,解得BP =33,从而AD =CE =2CP =2√BC 2−BP 2=2√432−332=4√190,故m +n =194,(D)正确.18.令f 是一个定义在正有理数集合上的函数,它具有性质:对于所有的正有理数a 和b ,f (ab )=f (a )+f (b ).假设f 还具有性质:对于每一个质数p ,f (p )=p .问以下哪个数x 满足f (x )<0?(A)1732(B)1116(C)79(D)76(E)2511解f (1)=f (1·1)=2f (1)⇒f (1)=0,f (1)=f (b ·1b )=f (b )+f (1b )=0⇒f (1b)=−f (b ),对于任意正有理数b ;f (a b )=f (a ·1b )=f (a )+f (1b)=f (a )−f (b ),对于任意正有理数a 和b ;f (p n )=f (p ·p ·····p n 个)=nf (p ),对于任意质数p ,n ∈N .因此,f (2511)=f (25)−f (11)=2f (5)−f (11)=2·5−11=−1<0,故(E)正确.19.由x 2+y 2=3|x −y |+3|x +y |的图像所界定的图形的面积是m +nπ,其中m 和n 是整数.问m +n 是多少?(A)18(B)27(C)39(D)45(E)54解如图所示,对点(x,y )的位置进行讨论:(1)在第一象限:当y x 时,方程为x 2+y 2=3(x −y )+3(x +y ),化简可得(x −3)2+y 2=9,它是一个八分之一圆;当y >x 时,方程可化为x 2+(y −3)2=9,也是一个八分之一圆;(2)在第二象限:当y −x 时,方程可化为x 2+(y −3)2=9;当y <−x 时,方程可化为(x +3)2+y 2=9;(3)在第三象限:当y x 时,方程可化为(x +3)2+y 2=9;当y <x 时,方程可化为x 2+(y +3)2=9;(4)在第四象限:当y −x 时,方程可化为(x −3)2+y 2=9;当y <−x 时,方程可化为x 2+(y +3)2=9.综上可得,该图像由四个半径为3的半圆封闭而成,面积为62+2·π·32=36+18π,故m +n =36+18=54,(E)正确.20.数列1,2,3,4,5有多少种重新排列的方式,使得没有连续三项是递增的,也没有连续三项是递减的?(A)10(B)18(C)24(D)32(E)44解由题意可知,符合条件的数列中,连续两项的单调性只能是:增减增减,或减增减增.用排列表示即有:13254,14253,14352,15243,15342;21435,21534,23154,24153,24351,25143,25341;31425,31524,32415,32514,34152,34251,35142,35241.如果对集合{1,2,3,4,5}做一个置换1234554321 ,所得排列仍然符合条件,即以5,4开头的排列和以1,2开头的排列一样多.故所有符合条件的数列有(5+7)×2+8=32个,(D)正确.21.设ABCDEF 是等角六边形,由直线AB ,CD 和EF 所组成的三角形面积为192√3,由直线BC ,DE 和F A 所组成的三角形的面积是324√3.六边形ABCDEF 的周长可用m +n √p 表达,其中m ,n 和p 是正整数,并且p不能被任何质数的平方整除.问m +n +p 的值是多少?(A)47(B)52(C)55(D)58(E)63解如图所示,分别向两边延长各边,交于点G ,H ,I ,J ,K ,L ,由题意可得,S ∆GHI =192√3,S ∆JKL =324√3.由于六边形的每个内角都是120◦,因此图中所有三角形的内角都是60◦,即所有三角形都是等边三角形.于是,S ∆GHI =√34IG 2=√34(a +b +f )2=192√3,解得a +b +f =16√3;同理S ∆JKL =√34(c +d +e )2=324√3,得c +d +e =36.故六边形的周长为a +b +c +d +e +f =36+16√3,即m +n +p =36+16+3=55,(C)正确.22.Hiram 的代数笔记有50页,打印在25张纸上;第一张纸包括第1和第2页,第二张纸包括第3和第4页,以此类推.有一天,他去午餐前把笔记本放在桌子上,室友决定从笔记中间借几页.当Hiram回来时,他发现他的室友从笔记中拿走了连续的若干张纸,并且所有剩余纸张上页码的平均值正好是19.问有多少张纸被借走了?(A)10(B)13(C)15(D)17(E)20解设笔记被借走了x张纸,分别是从第a张纸到第a+x−1张纸,其中a+x−1 25,页码正好是从2a−1到2(a+x−1),页码和为(2a−1)+2a+···+2(a+x−1)=(2a−1)+2(a+x−1)2·2x=(4a+2x−3)x,而所有页码和为1+2+···+50=1275,从而剩下的页码和为19(50−2x)=1275−(4a+2x−3)x,整理得(4a+2x−41)x=325,解得x=13,a=10,故(B)正确.23.青蛙Frieda在一个3×3的方格表上开始一系列跳跃,每次跳跃都随机选择一个方向—–向上、向下、向左或向右,从一个方格移动到旁边的方格.她不能斜着跳,当跳跃的方向会使得Frieda离开方格表时,她会“绕个圈”,跳到相对的另一边.例如,如果Frieda从中心方格开始,向上跳跃两次,第一次跳跃后她将位于最上面一行的中间方格,第二次跳跃将使得Frieda跳到相对的边,落在最下面一行的中间方格.假设Frieda从中心方格出发,最多随机跳跃四次,并且当到达角落方格时就停止跳跃.问她在四次跳跃中到达角落方格的概率是多少?(A)916(B)58(C)37(D)2532(E)1316解记P(n)为第n次到达角落方格的概率,显然P(1)=0;第一次跳跃可朝四个方向,不妨设第一次跳跃向右,则当第二次跳跃向上或向下时,可到达角落方格,概率为24,即P(2)=12;若第二次跳跃向左,概率为14,即回到出发点S,此时第四次跳跃到达角落方格的概率为1 4×P(2)=18;若第二次跳跃向右,概率为14,此时到了S左边的方格,则第三次跳跃向上或向下可到达角落方格,即P(3)=14×12=18;若第三次跳跃向左,概率为14,则回到S右边的方格,第四次跳跃向上或向下可到达角落方格,概率为14×14×12=132,从而P(4)=18+132=532.而其它情况均四次到达不了角落方格.故所求概率为4∑i=1P(i)=0+12+18+532=2532,(D)正确.24.设a是正实数,考虑由(x+ay)2=4a2和(ax−y)2=a2组成的四边形的内部.对所有的a>0而言,这个区域的面积怎样用a来表示?(A)8a2(a+1)2(B)4aa+1(C)8aa+1(D)8a2a2+1(E)8aa2+1解如图所示,四边形由四条直线y=−1ax±2,y=ax±a围成,由于斜率之积为−1,从而四边形是个矩形.设直线y=−1ax+2与x轴负方向的夹角为θ,则tanθ=1a,矩形的长为4cosθ,宽为2cosθ,即所求面积为S=8cos2θ.于是S=4·2cos2θ=4(1+cos2θ)=4(1+1−tan2θ1+tan2θ)=4·21+tan2θ=8·11+1a2=8a21+a2.故(D)正确.25.将3枚不可区分的红色筹码,3枚不可区分的蓝色筹码和3枚不可区分的绿色筹码分别放入3×3方格表的各个小方格中,使得无论是垂直方向还是水平方向,都没有两个相同颜色的筹码相邻,问共有多少种放法?(A)12(B)18(C)24(D)30(E)36解首先考虑中心方格,不妨设放入红色筹码,分两种情况:(1)其余两个红色筹码在一边,四个方向均可:那剩下6个方格只能按以下方式放入,∆放一种颜色,空格放另一种颜色.此时有4×2=8种放法;(2)其余两个红色筹码各在一边,即在对角线上,两个方向均可:那剩下6个方格也只能按以下方式放入,∆放一种颜色,空格放另一种颜色.此时有2×2=4种放法.综上,中心方格放入红色筹码,共有12种放法.如果放入蓝色筹码或绿色筹码,也是一样,故所有的放法有3×12=36种,(E)正确.。

美国数学竞赛2020年真题AMC10 A(真题加详解)

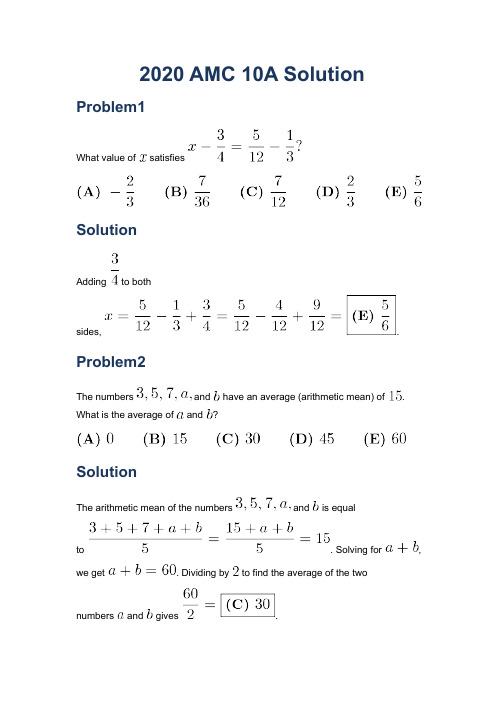
2020 AMC 10A Solution Problem1What value of satisfiesSolutionAdding to bothsides, . Problem2The numbers and have an average (arithmetic mean) of . What is the average of and ?SolutionThe arithmetic mean of the numbers and is equalto . Solving for , we get . Dividing by to find the average of the twonumbers and gives .Problem3Assuming , , and , what is the value in simplest form of the following expression?SolutionNote that is times .Likewise, is times and is times . Therefore, the product of the given fractionequals .Problem4A driver travels for hours at miles per hour, during which her cargets miles per gallon of gasoline. She is paid per mile, and her onlyexpense is gasoline at per gallon. What is her net rate of pay, in dollars per hour, after this expense?SolutionSince the driver travels 60 miles per hour and each hour she uses 2 gallons of gasoline, she spends $4 per hour on gas. If she gets $0.50 per mile, then she gets $30 per hour of driving. Subtracting the gas cost, her net rate of pay perhour is .Problem5What is the sum of all real numbers for whichSolution 1Split the equation into two cases, where the value inside the absolute value is positive and nonpositive.Case 1:The equation yields , which is equalto . Therefore, the two values for the positive caseis and .Case 2:Similarly, taking the nonpositive case for the value inside the absolute value notation yields . Factoring and simplifyinggives , so the only value for this case is .Summing all the values results in . Solution 2We have theequations and .Notice that the second is a perfect square with a double root at , and the first has real roots. By Vieta's, the sum of the roots of the first equationis .Problem6How many -digit positive integers (that is, integers between and , inclusive) having only even digits are divisible bySolutionThe ones digit, for all numbers divisible by 5, must be either or . However, from the restriction in the problem, it must be even, giving us exactly one choice () for this digit. For the middle two digits, we may choose any even integerfrom , meaning that we have total options. For the first digit, we follow similar intuition but realize that it cannot be , hence giving us 4 possibilities. Therefore, using the multiplication rule, weget . ~ciceroniiProblem7The integers from to inclusive, can be arranged to form a -by-square in which the sum of the numbers in each row, the sum of the numbers in each column, and the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum?SolutionWithout loss of generality, consider the five rows in the square. Each row must have the same sum of numbers, meaning that the sum of all the numbers in the square divided by is the total value per row. The sum of the integersis , and the common sum is .Solution 2Take the sum of the middle 5 values of the set (they will turn out to be the mean of each row). We get as our answer. ~BaolanProblem8What is the value ofSolution 1Split the even numbers and the odd numbers apart. If we group every 2 evennumbers together and add them, we get a total of . Summing the odd numbers is equivalent to summing the first 100 odd numbers, which is equal to . Adding these two, we obtain the answerof .Solution 2 (bashy)We can break this entire sum down into integer bits, in which the sum is , where is the first integer in this bit. We can find that the first sum of every sequence is , which we plug in for the bits in the entire sequenceis , so then we can plug it into the first term of every sequence equation we gotabove , and so the sum of every bit is , and we only found the value of , the sum of the sequenceis .-middletonkidsSolution 3Another solution involves adding everything and subtracting out what is not needed. The first step involvessolving. To do this, we can simply multiply and and divide by to getus . The next step involves subtracting out the numbers with minus signs. We actually have to do this twice, because we need to take out the numbers we weren’t supposed to add and then subtract them from the problem. Then, we can see that from to , incrementing by , there are numbers that we have to subtract. To do this we can do times divided by , and then we can multiply by , because we are counting by fours, not ones. Our answer will be , but remember, we have to do this twice. Once we do that,we will get . Finally, we just have to do , and our answer is .—Solution 4In this solution, we group every 4 terms. Our groups shouldbe: , ,, .... We add them together to get this expression: . This can be rewrittenas . We add this toget . ~BaolanSolution 5We can split up this long sum into groups of four integers. Finding the first few sums, we have that , , and . Notice that this is an increasing arithmetic sequence, with a common difference of . We can find the sum of the arithmetic sequence by finding the average of the first and last terms, and then multiplying by the number of terms in the sequence. The first term is , or , the last term is , or , and thereare or terms. So, we have that the sum of the sequenceis , or . ~Arctic_BunnySolution 3Taking the average of the first and last terms, and , we have that the mean of the set is . There are 5 values in each row, column or diagonal, so thevalue of the common sum is , or . ~Arctic_Bunny, edited by KINGLOGICProblem9A single bench section at a school event can hold either adults or children. When bench sections are connected end to end, an equal number of adults and children seated together will occupy all the bench space. What is the least possible positive integer value ofSolutionThe least common multiple of and is . Therefore, there mustbe adults and children. The total number of benchesis .Solution 2This is similar to Solution 1, with the same basic idea, but we don't need to calculate the LCM. Since both and are prime, their LCM must be theirproduct. So the answer would be . ~Baolan Problem10Seven cubes, whose volumes are , , , , , , and cubic units, are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top. Except for the bottom cube, the bottom face of each cube lies completely on top of the cube below it. What is the total surface area of the tower (including the bottom) in square units?Solution 1The volume of each cube follows the pattern of ascending, for isbetween and .We see that the total surface area can be comprised of three parts: the sides of the cubes, the tops of the cubes, and the bottom of the cube (which is just ). The sides areas can be measured as thesum , giving us . Structurally, if we examine the tower from the top, we see that it really just forms a square of area . Therefore, wecan say that the total surface area is . Alternatively, for the area of the tops, we could have found thesum , giving us as well.~ciceroniiSolution 2It can quickly be seen that the side lengths of the cubes are the integers from 1 to 7, inclusive.First, we will calculate the total surface area of the cubes, ignoring overlap. This valueis. Then, we need to subtract out the overlapped parts of the cubes. Between each consecutive pair of cubes, one of the smaller cube's faces is completely covered, along with an equal area of one of the larger cube's faces. The total area of theoverlapped parts of the cubes is thus equal to . Subtracting the overlapped surface area from the total surface area, weget . ~emerald_blockSolution 3 (a bit more tedious than others)It can be seen that the side lengths of the cubes using cube roots are all integers from to , inclusive.Only the cubes with side length and have faces in the surface area and the rest have . Also, since thecubes are stacked, we have to find the difference betweeneach and side length as ranges from to.We then come up withthis:.We then add all of this and get .Problem 11What is the median of the following list of numbersSolution 1We can see that is less than 2020. Therefore, there are ofthe numbers after . Also, there are numbers that are under and equal to . Since is equal to , it, with the other squares, willshift our median's placement up . We can find that the median of the whole set is , and gives us . Our answeris .~aryamSolution 2As we are trying to find the median of a -term set, we must find the average of the th and st terms.Since is slightly greater than , we know thatthe perfect squares through are less than , and the rest aregreater. Thus, from the number to the number , thereare terms. Since is less than and less than , we will only need to consider theperfect square terms going down from the th term, , after going down terms. Since the th and st terms areonly and terms away from the th term, we can simplysubtract from and from to get the two terms, whichare and . Averaging the two, weget ~emerald_blockSolution 3We want to know the th term and the th term to get the median. We know thatSo numbers are in between to .So the sum of and will result in , which means that is the th number.Also, notice that , which is larger than .Then the th term will be , and similarlythe th term will be .Solving for the median of the two numbers, we getProblem12Triangle is isoceles with .Medians and are perpendicular to each other,and . What is the area ofSolution 1Since quadrilateral has perpendicular diagonals, its area can be found as half of the product of the length of the diagonals. Also notethat has the area of triangle by similarity,so Thus,Solution 2 (Trapezoid)We know that , and since the ratios of its sidesare , the ratio of of their areas is .If is the area of , then trapezoid is the area of .Let's call the intersection of and . Let .Then . Since , and are heights of triangles and , respectively. Both of these triangles have base .Area ofArea ofAdding these two gives us the area of trapezoid , whichis .This is of the triangle, so the area of the triangleis ~quacker88, diagram by programjames1 Solution 3 (Medians)Draw median .Since we know that all medians of a triangle intersect at the incenter, we know that passes through point . We also know that medians of a triangle divide each other into segments of ratio . Knowing this, we can seethat , and since the two segments sumto , and are and , respectively.Finally knowing that the medians divide the triangle into sections of equal area, finding the area of is enough. .The area of . Multiplying this by givesus~quacker88Solution 4 (Triangles)We knowthat , , so .As , we can seethat and with a side ratio of .So , .With that, we can see that , and the area oftrapezoid is 72.As said in solution 1, .-QuadraticFunctions, solution 1 by ???Solution 5 (Only Pythagorean Theorem)Let be the height. Since medians divide each other into a ratio, and the medians have length 12, wehave and . From righttriangle ,so . Since is a median, . From righttriangle ,which implies . Bysymmetry .Applying the Pythagorean Theorem to righttriangle gives,so . Then the areaof isSolution 6 (Drawing)(NOT recommended) Transfer the given diagram, which happens to be to scale, onto a piece of a graph paper. Counting the boxes should give a reliable result since the answer choices are relatively far apart. -LingjunSolution 7Given a triangle with perpendicular medians with lengths and , the area will be .Solution 8 (Fastest)Connect the line segment and it's easy to seequadrilateral has an area of the product of its diagonals dividedby which is . Now, solving for triangle could be an option, but the drawing shows the area of will be less than the quadrilateral meaning the the area of is less than but greater than , leaving onlyone possible answer choice, .Problem 13A frog sitting at the point begins a sequence of jumps, where each jump is parallel to one of the coordinate axes and has length , and the direction of each jump (up, down, right, or left) is chosen independently at random. The sequence ends when the frog reaches a side of the square withvertices and . What is the probability that thesequence of jumps ends on a vertical side of the squareSolutionDrawing out the square, it's easy to see that if the frog goes to the left, it will immediately hit a vertical end of the square. Therefore, the probability of thishappening is . If the frog goes to the right, it will be in the center ofthe square at , and by symmetry (since the frog is equidistant from all sides of the square), the chance it will hit a vertical side of a square is . The probability of this happening is .If the frog goes either up or down, it will hit a line of symmetry along the corner it is closest to and furthest to, and again, is equidistant relating to the two closer sides and also equidistant relating the two further sides. The probability for it tohit a vertical wall is . Because there's a chance of the frog going up and down, the total probability for this case is and summing up all thecases,Solution 2Let's say we have our four by four grid and we work this out by casework. A is where the frog is, while B and C are possible locations for his second jump, while O is everything else. If we land on a C, we have reached the vertical side. However, if we land on a B, we can see that there is an equal chance of reaching the horizontal or vertical side, since we are symmetrically between them. So we have the probability of landing on a C is 1/4, while B is 3/4. Since C means that we have "succeeded", while B means that we have a half chance, wecompute .We get , or-yeskaySolution 3If the frog is on one of the 2 diagonals, the chance of landing on vertical or horizontal each becomes . Since it starts on , there is a chance (up,down, or right) it will reach a diagonal on the first jump and chance (left) it will reach the vertical side. The probablity of landing on a verticalis - Lingjun.Solution 4 (Complete States)Let denote the probability of the frog's sequence of jumps ends with ithitting a vertical edge when it is at . Note that by reflective symmetry over the line .Similarly, , and .Now we create equations for the probabilities at each of these points/states by considering the probability of going either up, down, left, or right from thatpoint:We have a system of equationsin variables, so we can solve for each of these probabilities. Plugging the second equation into the fourth equationgivesPlugging in the third equation into thisgivesNext, plugging in the second and third equation into the first equationyieldsNow plugging in (*) into this, wegetProblem14Real numbers and satisfy and . What is the value ofSolutionContinuing tocombineFrom the givens, it can be concluded that .Also, This meansthat . Substituting this informationinto , wehave . ~PCChess Solution 2As above, we need to calculate . Note that are the roots of andso and .Thuswhere and as in the previous solution. Thus the answer is .Solution 3Note that Now, we only need to find the values of andRecall that andthat We are able to solve thesecond equation, and doing so gets us Plugging this into the first equation, we getIn order to find the value of we find a common denominator so that we can add them together. This getsus Recallingthat and solving this equation, weget Plugging this into the first equation, wegetSolving the original equation, weget ~emerald_blockSolution 4 (Bashing)This is basically bashing using Vieta's formulas to find and (which I highly do not recommend, I only wrote this solution for fun).We use Vieta's to find a quadratic relating and . We set and to be the roots of the quadratic (because , and ). We can solve the quadratic to get theroots and . and are "interchangeable", meaning that it doesn't matter which solution or is, because it'll return the same result when plugged in. So we plug in for and andget as our answer.~BaolanSolution 5 (Bashing Part 2)This usually wouldn't work for most problems like this, but we're lucky that we can quickly expand and factor this expression in this question.We first change the original expression to ,because . This is equalto. We can factor andreduce to. Now our expression is just . Wefactor to get . So the answer would be .Solution 6 (Complete Binomial Theorem)We first simplify the expression to Then, we can solve for and given the system of equations in the problem.Since we can substitute for . Thus, this becomes theequation Multiplying both sides by , weobtain or By the quadratic formula we obtain . We also easily find thatgiven , equals the conjugate of . Thus, plugging our valuesin for and , our expression equalsBy the binomial theorem, we observe that every second terms of theexpansions and will cancel out (since a positive plus a negative of the same absolute value equals zero). We also observe that the other terms notcanceling out are doubled when summing the expansions of . Thus, our expression equals whichequals which equals .Problem15A positive integer divisor of is chosen at random. The probability that thedivisor chosen is a perfect square can be expressed as , where and are relatively prime positive integers. What is ?SolutionThe prime factorization of is . This yields a totalof divisors of In order to produce a perfect square divisor, there must be an even exponent for each number in the prime factorization. Note that and can not be in the prime factorization of a perfect square because there is only one of each in Thus, thereare perfect squares. (For , you can have , , , , , or 0 s, etc.) The probability that the divisor chosen is a perfect squareisProblem16A point is chosen at random within the square in the coordinate plane whose vertices are and . Theprobability that the point is within units of a lattice point is . (A point is a lattice point if and are both integers.) What is to the nearest tenthSolution 1DiagramDiagram by MathandSki Using AsymptoteNote: The diagram represents each unit square of thegiven square.SolutionWe consider an individual one-by-one block.If we draw a quarter of a circle from each corner (where the lattice points are located), each with radius , the area covered by the circles should be . Because of this, and the fact that there are four circles, we writeSolving for , we obtain , where with , we get , and from here, we simplify and seethat ~CrypthesTo be more rigorous, note that since if thenclearly the probability is greater than . This would make sure the above solution works, as if there is overlap with thequartercircles.Solution 2As in the previous solution, we obtain the equation , which simplifies to . Since is slightly more than , is slightly less than . We notice that is slightly morethan , so is roughly ~emerald_blockSolution 3 (Estimating)As above, we find that we need to estimate .Note that we can approximate andso .And so our answer is .Problem 17Define How many integers are there such that ?Solution 1Notice that is a product of many integers. We either need one factor to be 0 or an odd number of negative factors.Case 1: There are 100 integers for whichCase 2: For there to be an odd number of negative factors, must be between an odd number squared and an even number squared. This means that thereare total possible values of . Simplifying, thereare possible numbers.Summing, there are total possible values of . ~PCChess Solution 2Notice that is nonpositive when isbetween and , and , and (inclusive), whichmeans that the amount of valuesequals.This reducesto~ZericSolution 3 (end behavior)We know that is a -degree function with a positive leading coefficient. Thatis, .Since the degree of is even, its end behaviors match. And since theleading coefficient is positive, we know that both ends approach as goes in either direction.So the first time is going to be negative is when it intersects the -axis atan -intercept and it's going to dip below. This happens at , which is the smallest intercept.However, when it hits the next intercept, it's going to go back up again into positive territory, we know this happens at . And when it hits , it's going to dip back into negative territory. Clearly, this is going to continue to snake around the intercepts until .To get the amount of integers below and/or on the -axis, we simply need to count the integers. For example, the amount of integers in betweenthe interval we got earlier, we subtract and addone. integers, so there are four integers in this interval that produce a negative result.Doing this with all of the other intervals, we have. Proceed with Solution 2. ~quacker88Problem18Let be an ordered quadruple of not necessarily distinct integers, each one of them in the set For how many such quadruples is it truethat is odd? (For example, is one such quadruple, because is odd.)SolutionSolution 1 (Parity)In order for to be odd, consider parity. We must have (even)-(odd) or (odd)-(even). There are ways to pick numbers to obtain an even product. There are ways to obtain an odd product. Therefore, the total amount of ways to make oddis .-MidnightSolution 2 (Basically Solution 1 but more in depth)Consider parity. We need exactly one term to be odd, one term to be even. Because of symmetry, we can set to be odd and to be even, then multiply by If is odd, both and must be odd, therefore thereare possibilities for Consider Let us say that is even. Then there are possibilities for However, can be odd, in which case we have more possibilities for Thus there are ways forus to choose and ways for us to choose Therefore, also consideringsymmetry, we have total values ofSolution 3 (Complementary Counting)There are 4 ways to choose any number independently and 2 ways to choose any odd number independently. To get an even products, wecount: , which is . The number of ways to get an odd product can be counted like so: , which is , or . So, for oneproduct to be odd the other to be even: (order matters). ~ Anonymous and Arctic_BunnySolution 4 (Solution 3 but more in depth)We use complementary counting: If the difference is even, then we can subtract those cases. There are a total of cases.For an even difference, we have (even)-(even) or (odd-odd).From Solution 3:"There are 4 ways to choose any number independently and 2 ways to choose any odd number independently. even products:(number)*(number)-(odd)*(odd): . odd products: (odd)*(odd): ."With this, we easily calculate . Problem19As shown in the figure below, a regular dodecahedron (the polyhedron consisting of congruent regular pentagonal faces) floats in space with two horizontal faces. Note that there is a ring of five slanted faces adjacent to the top face, and a ring of five slanted faces adjacent to the bottom face. How many ways are there to move from the top face to the bottom face via a sequence of adjacent faces so that each face is visited at most once and moves are not permitted from the bottom ring to the top ring?DiagramSolution 1Since we start at the top face and end at the bottom face without moving from the lower ring to the upper ring or revisiting a face, our journey must consist of the top face, a series of faces in the upper ring, a series of faces in the lower ring, and the bottom face, in that order.We have choices for which face we visit first on the top ring. From there, we have choices for how far around the top ring we go before movingdown: or faces around clockwise, or faces aroundcounterclockwise, or immediately going down to the lower ring without visiting any other faces in the upper ring.We then have choices for which lower ring face to visit first (since every upper-ring face is adjacent to exactly lower-ring faces) and then once again choices for how to travel around the lower ring. We then proceed to the bottom face, completing the trip.Multiplying together all the numbers of choices we have, weget .Solution 2Swap the faces as vertices and the vertices as faces. Then, this problem is the same as 2016 AIME I #3which had an answerof .Problem20Quadrilateral satisfiesand Diagonals and intersect at point and What is the area of quadrilateralSolution 1 (Just Drop An Altitude)It's crucial to draw a good diagram for this one.Since and , we get . Now weneed to find to get the area of the whole quadrilateral. Drop an altitudefrom to and call the point of intersection . Let .Since , then . By dropping this altitude, we can also see two similar triangles, and .Since is , and , we get that . Now, if we redraw another diagram just of , we getthat . Now expanding, simplifying, and dividing by the GCF, we get . This factorsto . Since lengths cannot be negative, .Since , .So(I'm very sorry if you're a visual learner but now you have a diagram by ciceronii) ~ Solution by Ultraman~ Diagram by ciceroniiSolution 2 (Pro Guessing Strats)We know that the big triangle has area 300. Use the answer choices which would mean that the area of the little triangle is a multiple of 10. Thus the product of thelegs is a multiple of 20. Guess that the legs are equal to and , and because the hypotenuse is 20 we get . Testing small numbers, we get that when and , is indeed a square. The area of the triangle is thus 60, so the answer is .~tigershark22 ~(edited by HappyHuman)Solution 3 (coordinates)Let the pointsbe , , , ,and , respectively. Since lies on line , we know that . Furthermore, since , lies on the circle with diameter , so . Solving for and with these equations, we get the solutions and . We immediately discardthe solution as should be negative. Thus, we concludethat.Solution 4 (Trigonometry)Let and Using Law of Sineson we get and LoSon yieldsDivide the two toget Now,and solve the quadratic, taking the positive solution (C is acute) toget Soif then and By Pythagorean Theorem, and the answeris(This solution is incomplete, can someone complete it please-Lingjun) ok Latex edited by kc5170We could use the famous m-n rule in trigonometry in triangle ABC with Point E [Unable to write it here.Could anybody write the expression] We will find that BD is angle bisector of triangle ABC(because we will get tan (x)=1) Therefore by converse of angle bisector theorem AB:BC = 1:3. By using phythagorean theorem we have values of AB and AC. AB.AC = 120. Adding area of ABC and ACD Answer••360Problem21There exists a unique strictly increasing sequence of nonnegativeintegers suchthat What isSolution 1First, substitute with . Then, the given equationbecomes . Now consider only . Thisequals . Notethat equals , since the sum of a geometricsequence is . Thus, we can see that forms the sum of 17 different powers of 2. Applying the same method to eachof , , ... , , we can see that each of the pairs forms the sum of 17 different powers of 2. This gives us . But we must count also the term. Thus, Our answeris .~seanyoon777Solution 2(This is similar to solution 1) Let . Then, . The LHS canbe rewrittenas。

历届美国中学生数学竞赛试题及解答

历届美国中学生数学竞赛试题及解答
下面是近几届美国中学生数学竞赛试题及解答：
一、2019：
1、求解下列不等式（2 + 2·3² < 5·3）？
答案：2 + 2·3² < 5·3
2、如何快速计算三角形面积？
答案：三角形面积可以用公式：S=½·a·h，其中a为三角形的底边，h 为三角形高度。

二、2018：
1、求复数（1+i）³的模和辐角？
答案：复数（1+i）³的模为2，辐角为2π/3。

2、什么是余弦定理？
答案：余弦定理是一种三角形的定理，它规定了三角形的两条边的长度和它们之角之间的关系：C²=a² +b² -2ab·cosC。

三、2017：
1、如何快速求解圆面积？
答案：圆面积可以用公式：S=πr²，其中r为圆半径。

2、求解下列方程（x²-5x=3）？答案：x²-5x=3， x=2 或 3。

美国数学竞赛AMC8 -- 2005年真题解析(英文解析+中文解析)

美国数学竞赛AMC8 – 2005年真题解析(英文解析+中文解析)Problem 1Answer: BSolution:If x is the number, then 2x=60 and x=30. Dividing the number by 2 yields 15.中文解析：按照Connie的计算，这个数乘以2是60，可知这个数是30. 应该做的计算是30除以2，因而正确答案应该是15. 答案是B。

Problem 2Answer: CSolution:Karl paid 5*2.5=$12.5. 20% of this cost that he saved is 12.5*0.2=$2.5.中文解析：Karl按原价买了5个文件夹，支付的费用是：2.5*5=12.5. 折扣价是：1.25*0.8=10。

如果Karl 等一天，可以省2.5元。

答案是C.Problem 3Answer: DSolution:Rotating square ABCD counterclockwise 45° so that the line of symmetry BD is a vertical line makes it easier to see that 4 squares need to be colored to match its corresponding square.中文解析：如上图所示，以BD为对称轴，标蓝色的方块需要涂黑。

共4块，答案是D。

Problem 4Answer: CSolution:The perimeter of the triangle is 6.1+8.2+9.7=24cm. A square's perimeter is four times its side length, since all its side lengths are equal. If the square's perimeter is 24, the side length is24/4=6, and the area is 6*6=36.中文解析：三角形的周长是：6.1+8.2+9.7=24. 正方形的周长和三角形相等，也是24，则其边长是24/4=6. 其面积是：6*6=36. 答案是C。

AMC美国数学竞赛AMC.B 试题及答案解析

2003A M C10 B 1、Which of the following is the same as2、Al gets the disease algebritis and must take one green pill and one pink pill each day for two weeks. A green pill costs more than a pink pill, and Al’s pills cost a total of for the two weeks. How much does one green pill cost?3、The sum of 5 consecutive even integers is less than the sum of the ?rst consecutive odd counting numbers. What is the smallest of the even integers?4、Rose fills each of the rectangular regions of her rectangular flower bed with a different type of flower. The lengths, in feet, of the rectangular regions in her flower bed are as shown in the ?gure. She plants one flower per square foot in each region. Asters cost 1 each, begonias each, cannas 2 each, dahlias each, and Easter lilies 3 each. What is the least possible cost, in dollars, for her garden?5、Moe uses a mower to cut his rectangular -foot by -foot lawn. The swath he cuts is inches wide, but he overlaps each cut by inches tomake sure that no grass is missed. He walks at the rate of feet per hour while pushing the mower. Which of the following is closest to the number of hours it will take Moe to mow his lawn?.6、Many television screens are rectangles that are measured by the length of their diagonals. The ratio of the horizontal length to the height in a standard television screen is . The horizontal length of a “-inch” television screen is closest, in inches, to which of the following?7、The symbolism denotes the largest integer not exceeding . For example. , and . Compute.8、The second and fourth terms of a geometric sequence are and . Which of the following is a possible first term?9、Find the value of that satisfies the equation10、Nebraska, the home of the AMC, changed its license plate scheme. Each old license plate consisted of a letter followed by four digits. Each new license plate consists of three letters followed by three digits. By how many times is the number of possible license plates increased?11、A line with slope intersects a line with slope at the point . What is the distance between the -intercepts of these two lines?12、Al, Betty, and Clare split among them to be invested in different ways. Each begins with a different amount. At the end of one year they have a total of . Betty and Clare have both doubled their money, whereas Al has managed to lose . What was Al’s origin al portion?.13、Let denote the sum of the digits of the positive integer . For example, and . For how many two-digit values of is ?14、Given that , where both and are positive integers, find the smallest possible value for .15、There are players in a singles tennis tournament. The tournament is single elimination, meaning that a player who loses a match is eliminated. In the first round, the strongest players are given a bye, and the remaining players are paired off to play. After each round, the remaining players play in the next round. The match continues until only one player remains unbeaten. The total number of matches played is16、A restaurant offers three desserts, and exactly twice as many appetizers as main courses. A dinner consists of an appetizer, a main course, and a dessert. What is the least number of main courses that the restaurant should offer so that a customer could have a different dinner each night in the year ?.17、An ice cream cone consists of a sphere of vanilla ice cream and a right circular cone that has the same diameter as the sphere. If the ice cream melts, it will exactly ?ll the cone. Assume that the melted ice cream occupies of the volume of the frozen ice cream. What is the ratio of the cone’s height to its radius?18、What is the largest integer that is a divisor offor all positive even integers ?19、Three semicircles of radius are constructed on diameter of a semicircle of radius . The centers of the small semicircles divide into four line segments of equal length, as shown. What is the area of the shaded region that lies within the large semicircle but outside the smaller semicircles?20、In rectangle , and . Points and are on so that and . Lines and intersect at . Find the area of .21、A bag contains two red beads and two green beads. You reach into the bag and pull out a bead, replacing it with a red bead regardless of the color you pulled out. What is the probability that all beads in the bag are red after three such replacements?22、A clock chimes once at minutes past each hour and chimes on the hour according to the hour. For example, at 1 PM there is one chime and at noon and midnight there are twelve chimes. Starting at 11:15 AM on February , , on what date will the chime occur?23、A regular octagon has an area of one square unit. What is the area of the rectangle ?24、The ?rst four terms in an arithmetic sequence are , , , and, in that order. What is the ?fth term?25、How many distinct four-digit numbers are divisible by and have as their last two digits?。

1、下载文档前请自行甄别文档内容的完整性，平台不提供额外的编辑、内容补充、找答案等附加服务。
2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
3、如文档侵犯您的权益，请联系客服反馈,我们会尽快为您处理(人工客服工作时间：9:00-18:30)。

Solution
Answer: (C)
Lemma) if , then
The tedious algebra is left to the reader. (it is not bad at all)
Well, let us consider the cases where each of those step is definite (is never evaluate).
So, we have ,
--- (exception -> case 2)
--- (exception -> case 3)
--- (exception -> case 4)
--- (exception -> case 5)
If it is not any of the above 5 cases, then
if (--- exception -> case 6), then , ,
Hence, it is possible maximum of and minimum is 1.
2 possible combination of are and . Verification is left upto the reader. Right now, (C) is the most possible answer out of those 5.
Case 2) , then
Case 3) = 1, which is in the range.
Case 5) , hence
Case 6) Since , ,
Case 4) , this is quite an annoying special case. In this case, ,
is not define.
In this case,
and
Hence, and . Once,
you work out this system, you will get no solution with .
Thus, answer is (C).
SOLUTION 2:
After a bit of tedius algebra (that isn't too bad, but a little lengthy) we obtain
where ,
, , and
. In order for , we must have , ,
and . The first implies or , the second implies ,
, or , and the third implies or .
Since , in order to satisfy all 3 conditions we must have either or
. In the first case . For the latter case note that
so that and hence
. On the other hand so that
. Thus . Hence in any case the maximum
value for is while the minimum is (which can be achieved for instance when
or respectively). Hence the answer is .\\ \
Solution
From , we know that .
From the first inequality, we get . Subtracting
from this gives us , and thus .
Since must be an integer, it follows that .
Similarly, from the second inequality, we get . Again
subtracting from this gives us , or
. It follows from this that .
We now have a system of three equations: , , and
. Solving gives us and from this we find that
Since , we find that
.。