美国数学竞赛amc12
amc考试内容

amc考试内容
AMC(American Mathematics Competitions)是由美国数学协
会(MAA)主办的一系列数学竞赛。
该系列竞赛主要分为以下几个阶段:
1. AMC 8:面向6-8年级的学生,考察基础数学知识和解决问
题的能力。
考试时间为40分钟,共25道选择题。
2. AMC 10:面向10年级以下的学生,考察基础数学知识和解决问题的能力。
考试时间为75分钟,共25道选择题。
3. AMC 12:面向12年级以下的学生,考察更高级的数学知识和解决问题的能力。
考试时间为75分钟,共25道选择题。
4. AIME(American Invitational Mathematics Examination):AMC 10和AMC 12的前一部分考试,面向在AMC 10和
AMC 12中取得较高成绩的学生。
考试时间为3小时,共15
道填空题。
5. USAMO(United States of America Mathematics Olympiad):AMC和AIME的后一部分考试,面向在AIME中取得较高成
绩的学生。
考试时间为9小时,共6道证明题。
AMC考试内容包括基本数学知识、解答问题的能力、证明题
以及一些创造性问题,旨在培养学生的数学思维、问题解决能力和创新能力。
1996年美国数学竞赛-AMC_12

19961The addition below is incorrect.What is the largest digit that can be changed to make the addition correct?641852+9732456(A)4(B)5(C)6(D)7(E)8Each day Walter gets $3for doing his chores or $5for doing them exceptionally well.After 10days of doing his chores daily,Walter has received a total of $36.On how many days did Walter do them exceptionally well?A.3B.4C.5D.6E.7(3!)!3!=(A)1(B)2(C)6(D)40(E)120Six numbers from a list of nine integers are 7,8,3,5,and 9.The largest possible value of the median of all nine numbers in this list is(A)5(B)(C)7(D)8(E)Given that 0<a <b <c <d ,which of the following is the largest?A.a +b c +d B.a +d b +c C.b +c a +d D.b +d a +c E.c +d a +bIf f (x )=x (x +1)(x +2)(x +3)then f (0)+f (−1)+f (−2)+f (−3)=A.−8/9B.0C.8/9D.1E.10/9A father takes his twins and a younger child out to dinner on the twins’birthday.The restaurant charges $4.95for the father and $0.45for each year of a child’s age,where age is defined as the age at the most recent birthday.If the bill is $9.45,which of the following could be the age of the youngest child?A.1B.2C.3D.4E.5If 3=k ·2r and 15=k ·4r ,then r =(A)−log 25(B)log 52(C)log 105(D)log 25(E)52Triangle P AB and square ABCD are in perpendicular planes.Given that P A =3,P B =4,and AB =5,what is P D ?A.5B.√34C.√41D.2√13E.8This file was downloaded from the AoPS −MathLinks Math Olympiad Resources Page Page 1http://www.mathlinks.ro/1996How many line segments have both their endpoints located at the vertices of a given cube?(A)12(B)15(C)24(D)28(E)56Given a circle of raidus 2,there are many line segments of length 2that are tangent to the circle at their midpoints.Find the area of the region consisting of all such line segments.(A)π4(B)4−π(C)π2(D)π(E)2πA function f from the integers to the integers is defined as follows:f (x )= n +3if n is odd n/2if n is evenSuppose k is odd and f (f (f (k )))=27.What is the sum of the digits of k ?(A)3(B)6(C)9(D)12(E)15Sunny runs at a steady rate,and Moonbeam runs m times as fast,where m is a number greater than 1.If Moonbeam gives Sunny a head start of h meters,how many meters must Moonbeam run to overtake Sunny?(A)hm (B)h h +m (C)h m −1(D)hm m −1(E)h +m m −1Let E (n )denote the sum of the even digits of n .For example,E (5681)=6+8=14.Find E (1)+E (2)+E (3)+···+E (100).(A)200(B)360(C)400(D)900(E)2250Two opposite sides of a rectangle are each divided into n congruent segments,and the endpoints of one segment are joined to the center to form triangle A .The other sides are each divided into m congruent segments,and the endpoints of one of these segments are joined to the center to form triangle B .[See figure for n =5,m =7.]What is the ratio of the area of triangle A to the area of triangle B ?(A)1(B)m/n (C)n/m (D)2m/n (E)2n/mA fair standard six-sided dice is tossed three times.Given that the sum of the first two tosses equal the third,what is the probability that at least one ”2”is tossed?(A)16(B)91216(C)12(D)815(E)712In rectangle ABCD ,angle C is trisected by CF and CE ,where E is on AB ,F is on AD ,BE =6,and AF =2.Which of the following is closest to the area of the rectangle ABCD ?(A)110(B)120(C)130(D)140(E)150A circle of radius 2has center at (2,0).A circle of radius 1has center at (5,0).A line is tangent to the two circles at points in the first quadrant.Which of the following is closest to the y -intercept of the line?(A)√4(B)8/3(C)1+√(D)2√(E)3The midpoints of the sides of a regular hexagon ABCDEF are joined to form a smaller hexagon.What fraction of the area of ABCDEF is enclosed by the smaller hexagon?1996(A)12(B)√33(C)23(D)34(E)√32In the xy-plane,what is the length of the shortest path from (0,0)to (12,16)that does not go inside the circle (x −6)2+(y −8)2=25?(A)10√3(B)10√5(C)10√3+5π3(D)40√33(E)10+5πTriangles ABC and ABD are isosceles with AB =AC =BD ,and BD intersects AC at E .If BD is perpendicular to AC ,then ∠C +∠D is[img]/Forum/albump ic.php ?pic i d =537[/img ](A)115◦(B)120◦(C)130◦(D)135◦(E)not uniquely determinedFour distinct points,A ,B ,C ,and D ,are to be selected from 1996points evenly spaced around a circle.All quadruples are equally likely to be chosen.What is the probability that the chord AB intersects the chord CD?(A)14(B)13(C)12(D)23(E)34The sum of the lengths of the twelve edges of a rectangular box is 140,and the distance from one corner of the box to the farthest corner is 21.The total surface area of the box is(A)776(B)784(C)798(D)800(E)812The sequence 1,2,1,2,2,1,2,2,2,1,2,2,2,2,1,2,2,2,2,2,1,2,...consists of 1s separated by blocks of 2s with n 2s in the nth block.The sum of the first 1234terms of this sequence is(A)1996(B)2419(C)2429(D)2439(E)2449Given that x 2+y 2=14x +6y +6,what is the largest possible value that 3x +4y can have?(A)72(B)73(C)74(D)75(E)76An urn contains marbles of four colors:red,white,blue,and green.When four marbles are drawn without replacement,the following events are equally likely:(a)the selection of four red marbles;(b)the selection of one white and three red marbles;(c)the selection of one white,one blue,and two red marbles;and (d)the selection of one marble of each color.What is the smallest number of marbles satisfying the given condition?(A)19(B)21(C)46(D)69Consider two solid spherical balls,one centered at (0,0,212)with radius 6,and the other centeredat (0,0,1)with radius 92.How many points (x,y,z )with only integer coordinates (lattice points)are there in the intersection of the balls?(A)7(B)9(C)11(D)13(E)15On a 4×4×3rectangular parallelepiped,vertices A ,B ,and C are adjacent to vertex D .The perpendicular distance from D to the plane containing A ,B ,and C is closest to(A)1.6(B)1.9(C)2.1(D)2.7(E)2.91996If n is a positive integer such that 2n has 28positive divisors and 3n has 30positive divisors,then how many positive divisors does 6n have?(A)32(B)34(C)35(D)36(E)38A hexagon inscribed in a circle has three consecutive sides each of length 3and three consecutive sides each of length 5.The chord of the circle that divides the hexagon into two trapezoids,one with three sides each of length 3and the other with three sides each of length 5,has length equal to m n ,where m and n are relatively prime positive integers.Find m +n .(A)309(B)349(C)369(D)389(E)409。
2020年度美国数学竞赛AMC12 A卷(带答案)

AMC2020 AProblem 1Carlos took of a whole pie. Maria took one third of the remainder. What portion of the whole pie was left?Problem 2The acronym AMC is shown in the rectangular grid below with grid lines spaced unit apart. In units, what is the sum of the lengths of the line segments that form the acronym AMCProblem 3A driver travels for hours at miles per hour, during which her car gets miles per gallon of gasoline. She is paid per mile, and her only expense is gasoline at per gallon. What is her net rate of pay, in dollars per hour, after this expense?Problem 4How many -digit positive integers (that is, integersbetween and , inclusive) having only even digits are divisible byProblem 5The integers from to inclusive, can be arranged to form a -by- square in which the sum of the numbers in each row, the sum of the numbers in each column, and the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum?Problem 6In the plane figure shown below, of the unit squares have been shaded. What is the least number of additional unit squares that must be shaded so that the resulting figure has two lines of symmetryProblem 7Seven cubes, whose volumes are , , , , , ,and cubic units, are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top. Except for the bottom cube, the bottom face of each cube lies completely on top of the cube below it. What is the total surface area of the tower (including the bottom) in square units?Problem 8What is the median of the following list of numbersProblem 9How many solutions does the equation have on the intervalProblem 10There is a unique positive integer suchthat What is the sum of the digits ofProblem 11A frog sitting at the point begins a sequence of jumps, where each jump is parallel to one of the coordinate axes and has length , and the direction of each jump (up, down, right, or left) is chosen independently at random. The sequence ends when the frog reaches a side of the square withvertices and . What is the probability that the sequence of jumps ends on a vertical side of the squareProblem 12Line in the coordinate plane has the equation . This line is rotated counterclockwise about the point to obtain line . What is the -coordinate of the -intercept of lineProblem 13There are integers , , and , each greater than 1, suchthat for all . What is ?Problem 14Regular octagon has area . Let be the area of quadrilateral . What isProblem 15In the complex plane, let be the set of solutions to and let be the set of solutions to . What is the greatest distance between a point of and a point ofA point is chosen at random within the square in the coordinate plane whose vertices are and . The probability that the point is within units of a lattice point is .(A point is a lattice point if and are both integers.) What is to the nearest tenthProblem 17The vertices of a quadrilateral lie on the graph of , and the -coordinates of these vertices are consecutive positive integers.The area of the quadrilateral is . What is the -coordinate of the leftmost vertex?Problem 18Quadrilateral satisfies , and . Diagonals and intersect at point ,and . What is the area of quadrilateral ?There exists a unique strictly increasing sequence of nonnegativeintegers such thatWhat isProblem 20Let be the triangle in the coordinate plane withvertices , , and . Consider the following five isometries (rigid transformations) of the plane: rotations of , , and counterclockwise around the origin, reflection across the -axis, and reflection across the -axis. How many ofthe sequences of three of these transformations (not necessarily distinct) will return to its original position? (For example, a rotation, followed by a reflection across the -axis, followed by a reflection across the -axis will return to its original position, but a rotation, followed by a reflection across the-axis, followed by another reflection across the -axis will not return to its original position.)How many positive integers are there such that is a multiple of , and the least common multiple of and equals times the greatest common divisor of andProblem 22Let and be the sequences of real numbers suchthat for all integers , where . WhatisProblem 23Jason rolls three fair standard six-sided dice. Then he looks at the rolls and chooses a subset of the dice (possibly empty, possibly all three dice) to reroll. After rerolling, he wins if and only if the sum of the numbers face up on the three dice is exactly . Jason always plays to optimize his chances of winning. What is the probability that he chooses to reroll exactly two of the dice?Suppose that is an equilateral triangle of side length , with the property that there is a unique point inside the triangle such that , , and . What isProblem 25The number , where and are relatively prime positive integers, has the property that the sum of all realnumbers satisfying is , where denotes the greatest integer less than or equal to and denotes the fractional part of . What is2020 AMC 12A Answer Key 1. C2. C3. E4. B5. C6. D7. B8. C9. E10.E11.B12.B13.B14.B15.D16.B17.D18.D19.C20.A21.D22.B23.A24.B25.C。
全美数学竞赛流程

全美数学竞赛流程全美数学竞赛(AMC)是美国数学协会(MAA)主办的一项数学竞赛,分为AMC 10和AMC 12两个级别。
以下是全美数学竞赛的流程:1. 注册报名:学生可以通过学校或个人报名参加AMC竞赛。
报名通常在每年10月开始,截止日期一般在11月初。
2. 竞赛日期:竞赛通常分为两个日期进行,AMC 10和AMC12的日期可能不同。
竞赛通常在每年2月进行,考试时间为75分钟。
3. 考试内容:AMC竞赛分为多个选择题,每个竞赛级别有25道题目。
AMC 10适用于初中和低年级高中学生,AMC 12适用于高年级高中学生。
题目类型包括代数、几何、概率、数论等数学知识点。
4. 答题方式:学生需要在答题卡上选择正确答案。
答题卡需要填写个人信息和参加的竞赛级别。
5. 判题与分数:竞赛结束后,答题卡会被寄回MAA进行判题。
每道题目的正确答案会公布在MAA网站上。
学生可以在个人平台上查询自己的分数和排名。
6. 参赛资格:根据竞赛成绩,学生有机会获得进入AMC竞赛的更高级别,如AIME(AMC的随机选取的约5%到接近2.5%的学生进入AIME竞赛)。
7. 成绩认可:AMC竞赛成绩被广泛用于许多数学竞赛和数学奖学金的选拔,包括全国数学奥林匹克、AMC奖学金、美国数学学会奖学金等。
8. 破解讲座:MAA常常会组织一些破解讲座,分享一些解题技巧和策略,帮助学生提高竞赛成绩和解题水平。
以上是全美数学竞赛的一般流程,具体流程可能会有一些差异,可以参考MAA的官方网站或者咨询相关责任人了解更多详细信息。
amc数学竞赛成绩划分

amc数学竞赛成绩划分摘要:一、前言二、AMC 数学竞赛简介1.竞赛背景2.竞赛级别与分类三、AMC 数学竞赛成绩划分1.等级划分2.评分标准四、成绩划分的影响1.对参赛者的影响2.对数学教育的影响五、总结正文:一、前言近年来,AMC 数学竞赛在我国逐渐受到关注,吸引了大量学生参与。
对于这样一项具有国际影响力的数学竞赛,了解其成绩划分规则具有重要意义。
本文将对AMC 数学竞赛成绩划分的相关内容进行详细介绍。
二、AMC 数学竞赛简介AMC,全称American Mathematics Competitions,是美国数学竞赛的简称。
该竞赛起源于1950 年,目前已经成为全球范围内最具影响力的数学竞赛之一。
AMC 竞赛分为多个级别,包括AMC8、AMC10、AMC12、AIME (美国数学邀请赛)等,分别针对不同年龄段的学生。
三、AMC 数学竞赛成绩划分1.等级划分AMC 数学竞赛的成绩主要分为以下几个等级:(1)杰出奖(Distinction):成绩在前1% 的考生;(2)优秀奖(Merit):成绩在前10% 的考生;(3)达标奖(Pass):成绩在参赛者总数的50% 之前的考生;(4)未达标(Fail):成绩在参赛者总数的50% 之后的考生。
2.评分标准AMC 数学竞赛的评分标准主要依据考生的答题正确率。
在竞赛中,每道题目分值不同,难度也有所区别。
考生在规定时间内完成答题后,根据答对的题目数量和题目难度计算得分。
最终,根据得分情况对考生进行等级划分。
四、成绩划分的影响1.对参赛者的影响AMC 数学竞赛成绩的划分对参赛者具有激励作用,能够激发学生的学习兴趣和竞争意识。
同时,成绩划分也为参赛者提供了自我评价和定位的依据,有助于他们明确自己的优势和不足,调整学习策略。
2.对数学教育的影响AMC 数学竞赛成绩的划分对我国数学教育产生了一定的影响。
一方面,AMC 竞赛的成绩可以作为选拔优秀学生的参考依据;另一方面,AMC 竞赛的题目和理念对课堂教学具有启示作用,有助于提高数学教育的质量。
1990年美国数学竞赛-AMC_12

(A) 19 (B) 21 (C) 23 (D) 199 (E) 201
14 An acute isosceles triangle, ABC is inscribed in a circle. Through B and C, tangents to the circle are drawn, meeting at point D. If ∠ABC = ∠ACB = 2∠D and x is the radian measure of ∠A, then x =
1. Start X at 3 and S at 0 2. Increase the value of X by 2. 3. Increase the value of S by the value of X. 4. If S is at least 10000, then go to instsruction 5; otherwise, go to instruction 2 and proceed from there. 5. Print the value of X. 6. Stop.
then a =√ 2− 2
(A) 2
1 (B)
2
√ (C) 2 − 2
√ 2
(D) 2
√ 2+ 2 (E)
2
13 If the following instructions are carried out by a computer, which of X will be printed because of instruction 5?
[img]/Forum/albumpic.php?picid = 766sid = f 81d5c4216223342f e57ce
3 (A) π
AMC12美国数学竞赛2012-2014

AMC12 2014AProblem 1What isSolutionAt the theater children get in for half price. The price for adult tickets and child tickets is . How much would adult tickets and child tickets cost?SolutionWalking down Jane Street, Ralph passed four houses in a row, each painted a different color. He passed the orange house before the red house, and he passed the blue house before the yellow house. The blue house was not next to the yellow house. How many orderings of the colored houses are possible?SolutionSuppose that cows give gallons of milk in days. At this rate, how many gallons of milk will cows give in days?SolutionOn an algebra quiz, of the studentsscored points, scored points, scored points, and the rest scored points. What is the difference between the mean and median score of the students' scores on this quiz?SolutionThe difference between a two-digit number and the number obtained by reversing its digits is times the sum of the digits of either number. What is the sum of the two digit number and its reverse?SolutionThe first three terms of a geometric progression are , , and . What is the fourth term?SolutionA customer who intends to purchase an appliance has three coupons, only one of which may be used:Coupon 1: off the listed price if the listed price is at leastCoupon 2: dollars off the listed price if the listed price is at leastCoupon 3: off the amount by which the listed price exceedsFor which of the following listed prices will coupon offer a greater price reduction than either coupon or coupon ?Five positive consecutive integers starting with have average . What is the average of consecutive integers that start with ?SolutionThree congruent isosceles triangles are constructed with their bases on the sides of an equilateral triangle of side length . The sum of the areas of the three isosceles triangles is the same as the area of the equilateral triangle. What is the length of one of the two congruent sides of one of the isosceles triangles?SolutionDavid drives from his home to the airport to catch a flight. He drives miles in the first hour, but realizes that he will be hour late if he continues at this speed. He increases his speed by miles per hour for the rest of the way to the airport and arrives minutes early. How many miles is the airport from his home?SolutionTwo circles intersect at points and . The minor arcs measure on one circle and on the other circle. What is the ratio of the area of the larger circle to the area of the smaller circle?A fancy bed and breakfast inn has rooms, each with a distinctive color-coded decor. One day friends arrive to spend the night. There are no other guests that night. The friends can room in any combination they wish, but with no morethan friends per room. In how many ways can the innkeeper assign the guests to the rooms?SolutionLet be three integers such that is an arithmetic progressionand is a geometric progression. What is the smallest possible value of ?SolutionA five-digit palindrome is a positive integer with respective digits , where is non-zero. Let be the sum of all five-digit palindromes. What is the sum of the digits of .SolutionThe product , where the second factor has digits, is an integer whose digits have a sum of . What is ?SolutionA rectangular box contains a sphere of radius and eight smaller spheres of radius . The smaller spheres are each tangent to three sides of the box, and the larger sphere is tangent to each of the smaller spheres. What is ?SolutionThe domain of the function is an interval of length , where and are relatively prime positive integers. What is ?SolutionThere are exactly distinct rational numbers such that andhas at least one integer solution for . What is ?SolutionIn , , , and . Points and lieon and respectively. What is the minimum possible valueof ?SolutionFor every real number , let denote the greatest integer not exceeding , and let The set of all numbers suchthat and is a union of disjoint intervals. What is the sum of the lengths of those intervals?SolutionThe number is between and . How many pairs ofintegers are there such that andSolutionThe fraction where is the length of the period of the repeating decimal expansion. What is the sum ?SolutionLet , and for , let . For how many values of is ?The parabola has focus and goes through the points and . For how many points with integer coefficients is it truethat ?AMC 12 2013AProblem 1Square has side length . Point is on , and the areaof is . What is ?SolutionA softball team played ten games, scoring , and runs. They lost by one run in exactly five games. In each of the other games, they scored twice as many runs as their opponent. How many total runs did their opponents score?SolutionA flower bouquet contains pink roses, red roses, pink carnations, and red carnations. One third of the pink flowers are roses, three fourths of the red flowers are carnations, and six tenths of the flowers are pink. What percent of the flowers are carnations?SolutionWhat is the value ofSolutionTom, Dorothy, and Sammy went on a vacation and agreed to split the costs evenly. During their trip Tom paid $, Dorothy paid $, and Sammy paid $. In order to share the costs equally, Tom gave Sammy dollars, and Dorothy gave Sammy dollars. What is ?SolutionIn a recent basketball game, Shenille attempted only three-point shots andtwo-point shots. She was successful on of her three-point shots and of her two-point shots. Shenille attempted shots. How many points did she score?SolutionThe sequence has the property that every term beginning with the third is the sum of the previous two. That is,Suppose that and . What is ?SolutionGiven that and are distinct nonzero real numbers such that , what is ?SolutionIn , and . Points and are onsides , , and , respectively, such that and are parallelto and , respectively. What is the perimeter of parallelogram ?SolutionLet be the set of positive integers for which has the repeating decimal representation with and different digits. What is the sum of the elements of ?SolutionTriangle is equilateral with . Points and are on and points and are on such that both and are parallel to . Furthermore, triangle and trapezoids and all have the same perimeter. What is ?SolutionThe angles in a particular triangle are in arithmetic progression, and the side lengths are . The sum of the possible values of x equals where , and are positive integers. What is ?SolutionLet points and .Quadrilateral is cut into equal area pieces by a line passing through . This line intersects at point , where these fractions are in lowest terms. What is ?SolutionThe sequence, , , ,is an arithmetic progression. What is ?SolutionRabbits Peter and Pauline have three offspring—Flopsie, Mopsie, and Cotton-tail. These five rabbits are to be distributed to four different pet stores so that no store gets both a parent and a child. It is not required that every store gets a rabbit. In how many different ways can this be done?Solution, , are three piles of rocks. The mean weight of the rocks in is pounds, the mean weight of the rocks in is pounds, the mean weight of the rocks in the combined piles and is pounds, and the mean weight of the rocks in the combined piles and is pounds. What is the greatest possible integer value for the mean in pounds of the rocks in the combined piles and ?SolutionA group of pirates agree to divide a treasure chest of gold coins among themselves as follows. The pirate to take a share takes of the coins that remain in the chest. The number of coins initially in the chest is the smallest number for which this arrangement will allow each pirate to receive a positive whole number of coins. How many coins does the pirate receive?SolutionSix spheres of radius are positioned so that their centers are at the vertices of a regular hexagon of side length . The six spheres are internally tangent to a larger sphere whose center is the center of the hexagon. An eighth sphere is externally tangent to the six smaller spheres and internally tangent to the larger sphere. What is the radius of this eighth sphere?SolutionIn , , and . A circle with center andradius intersects at points and . Moreover and have integer lengths. What is ?SolutionLet be the set . For , define to mean thateither or . How many ordered triples of elements of have the property that , , and ?SolutionConsider. Which of the following intervals contains ?SolutionA palindrome is a nonnegative integer number that reads the same forwards and backwards when written in base 10 with no leading zeros. A 6-digit palindrome is chosen uniformly at random. What is the probability that is also a palindrome?Solutionis a square of side length . Point is on such that . The square region bounded by is rotated counterclockwise with center , sweeping out a region whose area is , where , , and are positive integers and . What is ?SolutionThree distinct segments are chosen at random among the segments whoseend-points are the vertices of a regular 12-gon. What is the probability that the lengths of these three segments are the three side lengths of a triangle with positive area?SolutionLet be defined by . How many complexnumbers are there such that and both the real and the imaginary parts of are integers with absolute value at most ?AMC12 2012AProblem 1A bug crawls along a number line, starting at . It crawls to , then turns around and crawls to . How many units does the bug crawl altogether?SolutionCagney can frost a cupcake every seconds and Lacey can frost a cupcake every seconds. Working together, how many cupcakes can they frostin minutes?SolutionA box centimeters high, centimeters wide, and centimeters long canhold grams of clay. A second box with twice the height, three times the width, and the same length as the first box can hold grams of clay. What is ?SolutionIn a bag of marbles, of the marbles are blue and the rest are red. If the number of red marbles is doubled and the number of blue marbles stays the same, what fraction of the marbles will be red?SolutionA fruit salad consists of blueberries, raspberries, grapes, and cherries. The fruit salad has a total of pieces of fruit. There are twice as many raspberries as blueberries, three times as many grapes as cherries, and four times as many cherries as raspberries. How many cherries are there in the fruit salad?SolutionThe sums of three whole numbers taken in pairs are , , and . What is the middle number?SolutionMary divides a circle into sectors. The central angles of these sectors, measured in degrees, are all integers and they form an arithmetic sequence. What is the degree measure of the smallest possible sector angle?SolutionAn iterative average of the numbers , , , , and is computed in the following way. Arrange the five numbers in some order. Find the mean of the first two numbers, then find the mean of that with the third number, then the mean of that with the fourth number, and finally the mean of that with the fifth number. What is the difference between the largest and smallest possible values that can be obtained using this procedure?SolutionA year is a leap year if and only if the year number is divisible by (such as ) or is divisible by but not by (such as ). The anniversary of the birth of novelist Charles Dickens was celebrated on February , , a Tuesday. On what day of the week was Dickens born?SolutionA triangle has area , one side of length , and the median to that side of length . Let be the acute angle formed by that side and the median. Whatis ?SolutionAlex, Mel, and Chelsea play a game that has rounds. In each round there is a single winner, and the outcomes of the rounds are independent. For each round the probability that Alex wins is , and Mel is twice as likely to win as Chelsea. What is the probability that Alex wins three rounds, Mel wins two rounds, and Chelsea wins one round?SolutionA square region is externally tangent to the circle withequation at the point on the side . Vertices and are on the circle with equation . What is the side length of this square?SolutionPaula the painter and her two helpers each paint at constant, but different, rates. They always start at , and all three always take the same amount of time to eat lunch. On Monday the three of them painted of a house, quittingat . On Tuesday, when Paula wasn't there, the two helpers paintedonly of the house and quit at . On Wednesday Paula worked by herself and finished the house by working until . How long, in minutes, was each day's lunch break?SolutionThe closed curve in the figure is made up of congruent circular arcs each of length , where each of the centers of the corresponding circles is among the vertices of a regular hexagon of side . What is the area enclosed by the curve?SolutionA square is partitioned into unit squares. Each unit square is painted either white or black with each color being equally likely, chosen independently and at random. The square is the rotated clockwise about its center, and every white square in a position formerly occupied by a black square is painted black. The colors of all other squares are left unchanged. What is the probability that the grid is now entirely black?SolutionCircle has its center lying on circle . The two circles meet at and . Point in the exterior of lies on circle and , ,and . What is the radius of circle ?SolutionLet be a subset of with the property that no pair of distinct elements in has a sum divisible by . What is the largest possible size of ?SolutionTriangle has , , and . Let denote the intersection of the internal angle bisectors of . What is ?SolutionAdam, Benin, Chiang, Deshawn, Esther, and Fiona have internet accounts. Some, but not all, of them are internet friends with each other, and none of them has an internet friend outside this group. Each of them has the same number of internet friends. In how many different ways can this happen?SolutionConsider the polynomialThe coefficient of is equal to . What is ?SolutionLet , , and be positive integers with such thatWhat is ?SolutionDistinct planes intersect the interior of a cube . Let be the unionof the faces of and let . The intersection of and consists of the union of all segments joining the midpoints of every pair of edges belonging to the same face of . What is the difference between the maximum and minimum possible values of ?SolutionLet be the square one of whose diagonals hasendpoints and . A point is chosen uniformly at random over all pairs of real numbers and suchthat and . Let be a translated copyof centered at . What is the probability that the square region determinedby contains exactly two points with integer coefficients in its interior?SolutionLet be the sequence of real numbers definedby , and in general,Rearranging the numbers in the sequence in decreasing order produces anew sequence . What is the sum of all integers , , such thatSolutionLet where denotes the fractional part of . Thenumber is the smallest positive integer such that the equationhas at least real solutions. What is ? Note: the fractional part of is a real number such that and is an integer.2014A1.C2.B3.B4.A5.C6.D7.A8.C9.B10.B11.C12.D13.B14.C15.B16.D17.A18.C19.E20.D21.A22.B23.B24.C25.B 2013A1. E2. C3. E4. C5. B6. B7. C8. D9. C10. D11. C12. A13. B14. B15. D16. E17. D18. B19. D20. B21. A22. E23. C24. E25. A2012A1. E2. D3. D4. C5. D6. D7. C8. C9. A10. D11. B12. D13. D14. E15. A16. E17. B18. A19. B20. B21. E22. C23. C24. C25. C。
amc数学竞赛成绩划分

amc数学竞赛成绩划分摘要:一、AMC数学竞赛简介二、AMC数学竞赛成绩划分及意义1.评分标准2.奖项设置3.全球排名三、如何提高AMC数学竞赛成绩1.学习策略2.解题技巧3.模拟练习四、总结正文:AMC数学竞赛是全球范围内最具影响力的数学竞赛之一,每年吸引着众多数学爱好者参加。
在我国,AMC竞赛同样具有很高的认可度和关注度。
本文将为大家介绍AMC数学竞赛的成绩划分及如何提高竞赛成绩。
一、AMC数学竞赛简介AMC数学竞赛分为两个阶段:AMC 8、AMC 10/12、AIME(美国数学邀请赛)。
参赛者需在规定时间内完成一定数量的数学题目。
题目难度逐渐递增,涵盖了从基础数学知识到高级数学技巧的各个方面。
二、AMC数学竞赛成绩划分及意义1.评分标准AMC数学竞赛采用积分制,正确答案得1分,部分正确或错误的答案不得分。
题目数量和分数决定了参赛者的总成绩。
2.奖项设置AMC竞赛设置有不同的奖项,如全球奖项、全国奖项、学校奖项等。
奖项的划分依据参赛者的总成绩在全球范围内的排名。
具体奖项设置如下:- 全球奖项:- 满分奖(Perfect Score):全球排名前1%- 荣誉奖(Honor Roll):全球排名前5%- 入围奖(Quick Start Award):全球排名前10%- 全国奖项:- 一等奖(First Place):全国排名前10名- 二等奖(Second Place):全国排名前20名- 三等奖(Third Place):全国排名前30名3.全球排名参赛者可以根据自己的成绩在全球排名中查找自己的位置。
全球排名有助于了解自己在国际范围内的数学水平,为今后的学术发展提供参考。
三、如何提高AMC数学竞赛成绩1.学习策略- 扎实掌握基础知识:AMC竞赛涉及的知识点较多,参赛者需要扎实掌握基础知识,才能在竞赛中游刃有余。
- 学习高级数学技巧:竞赛题目难度逐渐递增,掌握高级数学技巧对于解决难题至关重要。
- 总结经验:每次竞赛后,参赛者应总结自己的经验教训,找出不足之处,有针对性地进行提高。
2020年度美国数学竞赛AMC12A卷(带答案)
2020年度美国数学竞赛AMC12A卷(带答案)AMC2020 AProblem 1Carlos took of a whole pie. Maria took one third of the remainder. What portion of the whole pie was left?Problem 2The acronym AMC is shown in the rectangular grid below with grid lines spaced unit apart. In units, what is the sum of the lengths of the line segments that form the acronym AMCProblem 3A driver travels for hours at miles per hour, during which her car gets miles per gallon of gasoline. She is paid per mile, and her only expense is gasoline at per gallon. What is her net rate of pay, in dollars per hour, after this expense?Problem 4How many -digit positive integers (that is, integersbetween and , inclusive) having only even digits are divisible byProblem 5The integers from to inclusive, can be arranged to form a -by- square in which the sum of the numbers in each row, the sum of the numbers in each column, and the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum?Problem 6In the plane figure shown below, of the unit squares have been shaded. What is the least number of additional unit squares that must be shaded so that the resulting figure has two lines of symmetryProblem 7Seven cubes, whose volumes are , , , , , ,and cubic units, are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top. Except for the bottom cube, the bottom face of each cube lies completely on top of the cube below it. What is the total surface area of the tower (including the bottom) in square units?Problem 8What is the median of the following list of numbersProblem 9How many solutions does the equation have on the intervalProblem 10There is a unique positive integer suchthat What is the sum of the digits ofProblem 11A frog sitting at the point begins a sequence of jumps, where each jump is parallel to one of the coordinate axes and has length , and the direction of each jump (up, down, right, or left) is chosen independently at random. The sequence ends when the frog reaches a side of the square withvertices and . What is the probability that the sequence of jumps ends on a vertical side of the squareProblem 12Line in the coordinate plane has the equation . This line is rotated counterclockwise about the point to obtain line . What is the -coordinate of the -intercept of lineProblem 13There are integers , , and , each greater than 1, suchthat for all . What is ?Problem 14Regular octagon has area . Let be the area of quadrilateral . What isProblem 15In the complex plane, let be the set of solutions to and let be the set of solutions to . What is the greatest distance between a point of and a point ofA point is chosen at random within the square in the coordinate plane whose vertices are and . The probability that the point is within units of a lattice point is .(A point is a lattice point if and are both integers.) What is to the nearest tenthProblem 17The vertices of a quadrilateral lie on the graph of , andthe -coordinates of these vertices are consecutive positive integers. The area of the quadrilateral is . What isthe -coordinate of the leftmost vertex?Problem 18Quadrilateral satisfies , and . Diagonals and intersect at point ,and . What is the area of quadrilateral ?There exists a unique strictly increasing sequence of nonnegative integers suchthat What isProblem 20Let be the triangle in the coordinate plane withvertices , , and . Consider the following five isometries (rigid transformations) of the plane: rotations of , , and counterclockwise around the origin, reflection acrossthe -axis, and reflection across the -axis. How many ofthe sequences of three of these transformations (not necessarily distinct) will return to its original position? (For example, a rotation, followed by a reflection across the -axis, followed by a reflection across the -axis will return to its original position, but a rotation, followed by a reflection acrossthe -axis, followed by another reflection across the -axis will not return to its original position.)How many positive integers are there such that is a multiple of , and the least common multiple of and equals times the greatest common divisor of andProblem 22Let and be the sequences of real numbers suchthat for all integers , where . WhatisProblem 23Jason rolls three fair standard six-sided dice. Then he looks at the rolls and chooses a subset of the dice (possibly empty, possibly all three dice) to reroll. After rerolling, he wins if and only if the sum of the numbers face up on the three dice is exactly . Jason always plays to optimize his chances of winning. What is the probability that he chooses to reroll exactly two of the dice?Suppose that is an equilateral triangle of side length , with the property that there is a unique point inside the triangle such that , , and . What isProblem 25The number , where and are relatively prime positive integers, has the property that the sum of all realnumbers satisfying is , where denotes the greatest integer less than or equal to and denotes the fractional part of . What is2020 AMC 12A Answer Key 1. C2. C3. E4. B5. C6. D7. B8. C9. E10.E11.B12.B13.B14.B15.D16.B17.D18.D19.C20.A21.D22.B23.A24.B25.C。
2020年美国数学竞赛(AMC12A)的试题与解答

2020年美国数学竞赛(AMC12A)的试题与解答华南师范大学数学科学学院(510631)李湖南1.Carlos took 70%of a whole pie.Maria took one third of the remainder.What portion of the original pie was left?(A)10%(B)15%(C)20%(D)30%(E)35%译文卡洛斯取走了一整块派的70%,玛利亚取走了剩余的三分之一.问这块派还剩下多少?解(1−70%)×(1−13)=20%,故(C)正确.2.The acronym AMC is shown in the rectangular grid be-low with grid lines spaced 1unit apart.In units,what is the sum of the lengths of the line segments that form the acronym AMC?(A)17(B)15+2√2(C)13+4√2(D)11+6√2(E)21译文下图是矩形格子中的首字母缩写AMC ,其中小正方形格子的边长为1.则字母AMC 的线段长度之和是多少?解观察图形可知,直线段长度和为13,而小正方形对角线长度为√2,共4条,故总和为13+4√2,(C)正确.3.A driver travels for 2hours at 60miles per hour,during which her car gets 30miles per gallon of gasoline.She is paid $0.50per mile,and her only expense is gasoline at $2.00per gal-lon.What is her net rate of pay,in dollars per hour,after this expense?(A)20(B)22(C)24(D)25(E)26译文一位司机以60英里/小时的速度驾车2小时,她的车每跑30英里需要消耗1加仑汽油.她能获得0.50美元/英里的报酬,唯一的花费就是2美元/加仑的汽油.问她每小时除去消耗之后的净收益是多少美元?解1个小时她能跑60英里,获得60×0.50=30美元,汽油费为60÷30×2=4美元,故净收益为26美元,(E)正确.4.How many 4-digit positive integers (that is,integers be-tween 1000and 9999,inclusive)having only even digits are di-visible by 5?(A)80(B)100(C)125(D)200(E)500译文有多少个四位的正整数(也就是在1000和9999之间的整数)能被5整除且所有数字均为偶数?解依题意,符合条件的四位数的个位数只能是0,十位数和百位数可以是0,2,4,6,8,千位数只能是2,4,6,8,共有1×5×5×4=100种选择,故(B)正确.5.The 25integers from -10to 14,inclusive,can be arranged to form a 5-by-5square in which the sum of the numbers in each row,the sum of the numbers in each column,the sum of the num-bers along each of the main diagonals are all the same.What is the value of this common sum?(A)2(B)5(C)10(D)25(E)50译文将25个整数分别是从-10到14,放入5×5的格子中,使得格子里的每行、每列和两条对角线的数字和均相等.问这个数字和是多少?解这是一个5阶幻方问题,25个数字之和是(−10)+(−9)+···+13+14=50,分别放入5行,故每行的数字和是10,(C)正确.6.In the place figure shown below,3of the unit squares have been shaded.What is the least number of additional unit squares that must be shaded so that the resulting figure has two lines ofsymmetry?(A)4(B)5(C)6(D)7(E)8译文如下图所示,3个单元格被涂成阴影部分.问至少还需要把多少个单元格涂成阴影才能使整个图有两条对称轴?解由于这是一个4×5的矩形,两条对称轴只可能是长和宽两条边的中垂线,从而至少有7个单元格需要填涂,如右图所示.故(D)正确.7.Seven cubes,whose volumes are 1,8,27,64,125,216,and 343cubic units,are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top.Ex-cept for the bottom cube,the bottom face of each cube lies com-pletely on top of the cube below it.What is the total surface area of the tower (including the bottom)in square units?(A)644(B)658(C)664(D)720(E)749译文七个立方体,体积分别是1,8,27,64,125,216,343个立方单位,依次按照体积大小由底到顶垂直地堆积成一座塔.除了最底部的立方体,每个立方体的底面都完全被下面的立方体的顶面覆盖.问这座塔的表面积(包括底面)是多少个平方单位?解这七个数都是立方数,则这七个立方体的棱长分别是1,2,3,4,5,6,7,从而塔的侧面积为4×(12+22+...+72)=560,而上、下底面积之和为2×72=98,共658,故(B)正确.8.What is the median of the following list of 4040numbers?1,2,3,...,2020,12,22,32,...,20202(A)1974.5(B)1975.5(C)1976.5(D)1977.5(E)1978.5译文下列4040个数:1,2,3,...,2020,12,22,32,...,20202的中位数是多少?解由于442=1936,452=2025,从而以上数列按递增排列的话,就成为:1,12,...,4,22,...,1936,442,...,1976,1977,...,2020,452,462,...,20202此时,1976成为第2020个数,所求中位数为1976+19772=1976.5,故(C)正确.9.How many solutions does the equation tan (2x )=cos (x 2)have on the interval [0,2π]?(A)1(B)2(C)3(D)4(E)5译文方程tan (2x )=cos (x 2)在区间[0,2π]上有多少个解?解y =tan 2x 是一个周期为π2、值域为R 的函数,在一个周期(−π4,π4)内严格单调递增,y =cos x2是一个周期为4π、值域为[−1,1]的函数,在区间[0,2π]上严格单调递减.如图示,在区间(π4,3π4),(3π4,5π4),(5π4,7π4)内,这是y =tan 2x 完整的周期,两条曲线均有一个交点;在区间[0,π4),(7π4,2π]上,这是y =tan 2x 的半周期,两条曲线刚好也有一个交点.故共有5个交点,(E)正确.10.There is a unique integer n such that log 2(log 16n )=log 4(log 4n ).What is the sum of the digits of n ?(A)4(B)7(C)8(D)11(E)13译文存在唯一的整数n 使得log 2(log 16n )=log 4(log 4n )成立,则n 的各个数位上的数字之和是多少?解log 4(log 4n )=log 2(log 16n )=log 22(log 16n )2,可得log 4n =(log 16n )2,使用换底公式有ln n ln 4=(ln n ln 16)2=ln 2n4ln 24,从而ln n 4ln 4=1⇒n =44=256.故(E)正确.11.A frog sitting at the point (1,2)begins a sequence of jumps,where each jump is parallel to one of the coordinate ax-es and has length 1,and the direction of each jump (up,down,left,right)is chosen independently at random.The sequence ends when the frog reaches a side of the square with vertices (0,0),(0,4),(4,0),and (4,4).What is the probability that the sequence ofjumps ends on a vertical side of the square?(A)12(B)58(C)23(D)34(E)78译文一只青蛙坐在点(1,2)上,开始一系列的跳跃,每次跳跃都平行于坐标轴且长度为1,方向(上、下、左、右)是随机的且独立,当青蛙到达由点(0,0),(0,4),(4,0),(4,4)构成的正方形的一条边的时候,跳跃终止.问跳跃终止于正方形竖直的两条边上的概率是多少?解如图示,青蛙在点F 1处,它可以向四个方向跳跃,概率均为14,向左跳跃,立刻达成目标;向上、向右、向下分别跳跃到点A 1,C,A 3处,再通过其它跳跃达成目标.根据对称性,青蛙由点A 1,A 2,A 3,A 4出发达成目标的概率是一样的,设为a ;青蛙由点B 1,B 2出发达成目标的概率是一样的,设为b ;青蛙由点F 1,F 2出发达成目标的概率是一样的,设为x ;青蛙由点C 出发达成目标的概率设为c .因此,P (青蛙由F 1出发达成目标)=P (青蛙向左)+P (青蛙向上)×P (青蛙由A 1出发达成目标)+P (青蛙向右)×P (青蛙由C 出发达成目标)+P (青蛙向下)×P (青蛙由A 3出发达成目标),即有x =14+a 2+c 4;同理,可得方程组x =14+a 2+c 4a =14+x 4+b 4b =a 2+c 4c =x 2+b 2成立,解得(x,a,b,c )=(58,12,38,12).故(B)正确.12.Line l in the coordinate plane has equation 3x −5y +40=0.This line is rotated 45◦counterclockwise about the point (20,20)to obtain line k .What is the x –coordinate of the x –intercept of line k ?(A)10(B)15(C)20(D)25(E)30译文坐标平面上的直线l 的方程为3x −5y +40=0,其绕点(20,20)作逆时针旋转45◦后得到直线k .则直线k 与x 轴交点的横坐标是多少?解如图示,直线k 与l 的斜率分别为tan ∠1和tan ∠2,依题意有∠1=∠2+45◦,tan ∠2=35,于是tan ∠1=tan (∠2+45◦)=tan ∠2+tan 45◦1−tan ∠2·tan 45◦=4,从而得到直线k 的方程为y −20=4(x −20),当y =0时,求得x =15.故(B)正确.13.There are integer a,b and c ,each greater than 1,such that a √N b √N c √N =36√N 25for all N >1.What is b ?(A)2(B)3(C)4(D)5(E)6译文设a,b,c 均是大于1的整数,且式子a √N b √N c √N =36√N 25对于N >1均成立.问b 是多少?解a √Nb √N c√N =N 1a N 1ab N 1abc =N bc +c +1abc 36√N 25=N 2536,依题意可得,bc +c +1abc =2536,解得a =2,b =3,c =6.故(B)正确.14.Regular octagon ABCDEF GH has area n .Let m bethe area of quadrilateral ACEG .What is mn?(A)√24(B)√22(C)34(D)3√25(E)2√23译文设正八边形ABCDEF GH 的面积为n ,四边形ACEG 的面积为m .则m n是多少?解如图,取正八边形的中心点O ,连OA ,OB .令OA =a ,则AC=√2a,∠AOB =45◦,于是m =S ACEG =(√2a )2=2a 2,n =S ABCDEF GH =8S ∆AOB =8×12a 2sin 45◦=2√2a 2,从而得m n =2a 22√2a 2=√22.故(B)正确.15.In the complex plane,let A be the set of solutions toz 3−8=0and let B be the set of solutions to z 3−8z 2−8z +64=0.What is the greatest distance between a point of A and a point of B ?(A)2√3(B)6(C)9(D)2√21(E)9+√3译文在复平面上,设A 是方程z 3−8=0的解集,B 是方程z 3−8z 2−8z +64=0的解集.问A 中一点到B 中一点的最远距离是多少?解解方程z 3−8=(z −2)(z 2+2z +4)=0,得A ={2,−1+√3i,−1−√3i };解方程z 3−8z 2−8z +64=(z −8)(z 2−8)=0,得B ={8,2√2,−2√2}.容易看出A 到B 的最远距离为d = (−1+√3i )−8 =√(−9)2+√32=2√21.故(D)正确.16.A point is chosen at random within the square in the co-ordinate plane whose vertices are (0,0),(2020,0),(2020,2020),and (0,2020).The probability that the point lies within d units ofa lattice point is 12.(A point (x,y )is a lattice point if x and y areboth integers.)What is d to the nearest tenth?(A)0.3(B)0.4(C)0.5(D)0.6(E)0.7译文坐标平面上有一个以(0,0),(2020,0),(2020,2020)和(0,2020)为顶点的正方形.在正方形内随机选择一个点,该点位于格点的d 个单位内的概率是12.(点(x,y )称为格点,若x 和y 均为整数.)则d 精确到十分位是多少?解如图示,以格点为圆心,d 为半径作一些圆,则正方形内的圆内部分就是符合条件的点集.因此,该点落在此区域的概率为P=该区域的面积正方形面积,即12=(20192+2019×2+1)πd220202=πd2,求得d=√12π≈0.4,故(B)正确.17.The vertices of a quadrilateral lie on the graph of y= ln x,and the x-coordinates of these vertices are consecutive pos-itive integers.The area of the quadrilateral is ln 9190.What is thex-coordinate of the leftmost vertex?(A)6(B)7(C)10(D)12(E)13译文一个四边形的顶点均在y=ln x的图像上,且它们的横坐标是连续正整数.该四边形的面积为ln 9190,则最左边顶点的横坐标是多少?解如图示,ABCD 是y=ln x上的四边形,过A作x轴的平行线,过C作x轴的垂线,交于点P,连结P B,P D,设点A坐标为(x,ln x),则有B(x+1,ln(x+1)),C(x+2,ln(x+2)),D(x+3,ln(x+3)),P(x+2,ln x),于是S ABCD=S∆P AB+S∆P BC+S∆P CD−S∆P AD=12×2×[ln(x+1)−ln x]+2×12×[ln(x+2)−ln x]×1−12×2×[ln(x+3)−ln x]=ln (x+1)(x+2)x(x+3)=ln9190.可得(x+1)(x+2)x(x+3)=9190,解得x=12或x=−15(舍去).故(D)正确.18.Quadrilateral ABCD satisfies∠ABC=∠ACD= 90◦,AC=20and CD=30.Diagonals AC and BD inter-sects at point E and AE=5.What is the area of Quadrilateral ABCD?(A)330(B)340(C)350(D)360(E)370译文四边形ABCD满足∠ABC=∠ACD=90◦, AC=20,CD=30.对角线AC和BD交于点E,且AE=5.求四边形ABCD 的面积是多少?解如图示,以AC为直径作一个圆,交BD与点F,依题意可得EC=15,ED=√EC2+CD2=15√5.设BE=x,依据相交弦定理AE·EC=BE·EF,则得EF=75x,DF=15√5−75x,DB=15√5+x;再由切割线定理DC2=DF·DB,得900=(15√5−75x)·(15√5+x),解得x=3√5或x=−5√5(舍去).而S∆ACD=12×20×30=300,S∆ABCS∆ACD=BEED=15,可得S∆ABC=60,故S ABCD=360,(D)正确.19.There exists a unique strictly increasing sequence of nonnegative integers a1<a2<···<a k such that2289+1217+1= 2a1+2a2+···+2a k.What is k?(A)117(B)136(C)137(D)273(E)306译文存在唯一严格递增的非负整数列a1<a2<···< a k使得2289+1217+1=2a1+2a2+···+2a k,则k是多少?解令217=x,则2289+1217+1=x17+1x+1=x16−x15+x14−x13+...+x2−x+1,而x16−x15=2272−2255=2271+2270+...+2255,同理x14−x13=2238−2221=2237+2236+ (2221)...,x2−x=234−217=233+232+ (217)从而2289+1217+1=20+(217+···+232+233)+···+(2255+···+2270+2271),共8×17+1=137项,故(C)正确.20.Let T be the triangle in the coordinate plane with vertices (0,0),(4,0),and(0,3).Consider the following five isometries (rigid transformations)of the plane:rotation of90◦,180◦,and 270◦counterclockwise around the origin,reflection across the x-axis,and reflection across the y-axis.How many of the125 sequences of three of these transformations(not necessarily dis-tinct)will return T to its original position?(For example,a180◦rotation,followed by a reflection across the x-axis,followed by a reflection across the y-axis will return T to its original position, but a90◦rotation,followed by a reflection across the x-axis,fol-lowed by another reflection across the x-axis will not return T to its original position.)(A)12(B)15(C)17(D)20(E)25译文设T是坐标平面上以(0,0),(4,0)和(0,3)为顶点的三角形.考虑以下五种平面上的等距变换(刚体变换):绕原点作90◦,180◦和270◦的逆时针旋转,关于x轴或y轴的反射.任选三种变换(不必不同)可以组成125种组合,有多少种组合将使得T 变回起始位置?(例如,一个关于y 轴的反射,接着一个关于x 轴的反射,再接着一个180◦的旋转,将会使得T 变回起始位置;但一个关于x 轴的反射,接着另一个关于x 轴的反射,再接着一个90◦的旋转,将不会使得T 变回起始位置.)解分两种情况:(1)全部由旋转组成:只要三次旋转的角度和为360◦或720◦即可满足要求,因此有90◦+90◦+180◦,90◦+180◦+90◦,180◦+90◦+90◦,270◦+270◦+180◦,270◦+180◦+270◦,180◦+270◦+270◦共6种组合;(2)由旋转和反射组合而成:有y 轴+x 轴+180◦,y 轴+180◦+x 轴,180◦+x 轴+y 轴,180◦+y 轴+x 轴,x 轴+180◦+y 轴,x 轴+y 轴+180◦,也是6种组合.故(A)正确.21.How many positive integers n are there such that n is a multiple of 5,and the least common multiple of 5!and n equals 5times the greatest common divisor of 10!And n ?(A)12(B)24(C)36(D)48(E)72译文有多少个正整数n ,使得n 是一个5的倍数,且n 与5!的最小公倍数是n 与10!的最大公因数的5倍?解由题意,[n,5!]=5×(n,10!),而5!=23×3×5,10!=28×34×52×7,可知n 不含除2,3,5,7以外的素因子,可设n =2a ×3b ×5c ×7d ,其中a,b,c,d ∈N ,且c 1.根据[2a ×3b ×5c ×7d ,23×3×5]=5×(2a ×3b ×5c×7d,28×34×52×7),以及最大公因数和最小公倍数的取法,可得3 a 8,1 b 4,c =3,0 d 1.故n 有6×4×1×2=48种取法,(D)正确.22.Let (a n )and (b n )be the sequence of real numbers suchthat (2+i )n =a n +b n i for all integers n 0,where i =√−1.What is ∞∑n =0a n b n7n?(A)38(B)716(C)12(D)916(E)47译文设(a n )和(b n )是使(2+i )n =a n +b n i 对所有的整数n 0均成立的实数列,其中i =√−1,则∞∑n =0a n b n7n是多少?解由(2+i )n =a n +b n i ,可得(2−i )n =a n −b n i,两式相加减得(2+i )n +(2−i )n =2a n ,(2+i )n −(2−i )n =2b n i从而a nb n =(2+i )n +(2−i )n 2·(2+i )n −(2−i )n2i =(3+4i )n −(3−4i )n4i 于是∞∑n =0a n b n 7n =∞∑n =017n ·(3+4i )n −(3−4i )n4i =14i ·∞∑n =0(3+4i )n −(3−4i )n 7n=14i·11−3+4i 7−11−3−4i 7=14i ·(74−4i −74+4i )=716故(B)正确.23.Jason rolls three fair standard six-sided dice.Then he looks at the rolls and chooses a subset of the dice (possibly emp-ty,possibly all three dice)to reroll.After rerolling,he wins if and only if the sum of the numbers faces up on the three dice is exactly 7.Jason always plays to optimize his chances of winning.What is the probability that he chooses to reroll exactly two of the dice?(A)736(B)524(C)29(D)1772(E)14译文詹森掷3颗标准、均匀的骰子,他看了结果之后会选择若干(可能是0,也可能是3)颗重掷.当3颗骰子正面朝上的数字和为7点的时候,他就赢了.詹森总是按照朝着他赢的最优策略去掷.问他刚好选择2颗骰子重掷的概率是多少?解掷1颗骰子得1,2,3,4,5,6点的概率均为16;掷2颗骰子得3点只有两种情况:12和21,概率为236,···;掷3颗骰子得7点有15种情况:115,151,511,124,142,214,241,412,421,133,313,331,223,232,322,概率为15216,···.经过计算,所有结果如下表所示:分类/概率/结果1234567掷1颗161616161616掷2颗136236336436536掷3颗1216321632161021615216因此,詹森要选择2颗骰子重掷,则上次掷的结果中,任意两颗骰子的数字和不能小于7点,否则他将选择重掷1颗骰子;且不能3颗骰子都是4点或者以上,要不然他将选择2019年全国高中数学联赛一试A 卷第10题的探究广东省佛山市乐从中学(528315)林国红一、题目呈现题目(2019年全国高中数学联赛一试(A 卷)第10题)在平面直角坐标系xoy 中,圆Ω与抛物线Γ:y 2=4x 恰有一个公共点,且圆Ω与x 轴相切于抛物线Γ的焦点F ,求圆Ω的半径.二、解法探究解法1(利用均值不等式)由题可知抛物线Γ的焦点为F (1,0),由对称性,不妨设圆Ω在x 轴上方与x 轴相切于F ,设圆Ω的半径为r .故圆Ω的方程为(x −1)2+(y −r )2=r 21⃝将x =14y 2代入1⃝并化简,得(y 24−1)2+y 2−2ry =0.显然y >0,故r =y 4+8y 2+1632y =(y 2+4)232y2⃝根据条件,2⃝恰有一个正数解y ,该y 值对应Ω与Γ的唯一公共点.考虑f (y )=(y 2+4)232y(y >0)的最小值.重掷3颗骰子.根据以上分析,满足条件的情况有:(1)掷出1点、6点、6点,3种情况;(2)掷出2点、5点、5点,3种情况;(3)掷出2点、5点、6点,6种情况;(4)掷出2点、6点、6点,3种情况;(5)掷出3点、4点、4点,3种情况;(6)掷出3点、4点、5点,6种情况;(7)掷出3点、4点、6点,6种情况;(8)掷出3点、5点、5点,3种情况;(9)掷出3点、5点、6点,6种情况;(10)掷出3点、6点、6点,3种情况.由加法原理,共42种情况,故所求概率为42216=736,(A)正确.24.Suppose that ∆ABC is an equilateral triangle of side length s ,with the property that there is a unique point P insidethe triangle such that AP =1,BP =√3,CP =2.What is s ?(A)1+√2(B)√7(C)83(D)√5+√5(E)2√2译文设∆ABC 是一个边长为s 的正三角形,内部有一点P ,使得AP =1,BP =√3,CP =2.问s 是多少?解如图,将∆AP C 绕点A 逆时针旋转60◦,得到∆ADB ,连结DP ,则AD =AP =1,DB =P C =2,∠DAP =60◦,因而∆ADP 是一个正三角形,可得DP =1,进而DP 2+BP 2=DB 2,所以∆DP B 是一个直角三角形,∠DP B =90◦,因此∠AP B =150◦.根据余弦定理,s 2=AB 2=AP 2+P B 2−2AP ·P B ·cos ∠AP B =1+3−2√3cos 150◦=7,即得s =√7.故(B)正确.25.The number a =pq,where p and q are relatively prime positive integers,has the property that the sum of all real num-bers x satisfying ⌊x ⌋·{x }=a ·x 2is 420,where ⌊x ⌋denotes the greatest integer less than or equal to x and {x }=x −⌊x ⌋denotes the dfractional part of x .What is p +q ?(A)245(B)593(C)929(D)1331(E)1332译文数a =pq满足性质:符合方程⌊x ⌋·{x }=a ·x 2的所有实数x 之和为420,其中p ,q 是互素的正整数,⌊x ⌋表示小于等于x 的最大整数,{x }=x −⌊x ⌋表示x 的小数部分.则p +q 是多少?解设⌊x ⌋=n ,{x }=r ,则x =n +r,0 r <1,代入方程⌊x ⌋·{x }=a ·x 2,整理得ar 2+(2a −1)nr +an 2=0,解得r =(1−2a )n ±√(1−4a )n 22a ,可知0<a 14.再由0 r =(1−2a )n −√(1−4a )n 22a<1,解得0 n <2a (1−2a )−√1−4ac .若c 是整数,则∑x =∑(n +r )=∑(n +nc)=c −1∑n =0(n +n c )=c 2−12=420,解得c =29,从而a =29900;若c 不是整数,则∑x =⌊c ⌋∑n =0(n +n c )=⌊c ⌋(⌊c ⌋+1)2·c +1c=420c 无解.故a =29900,p +q =929,(C)正确.。
美国数学测评AMC12A-2019(中英双语)

2019AMC 12A Problems1. The area of a pizza with radius 4 is N percent larger than the area of a pizza with radius 3 inches. What is the integer closest to N ?半径为4英寸的比萨饼面积比半径为3英寸的比萨饼面积大百分之N 。
最接近N 的整数是什么?A B C D E ()25()33()44()66()782.Suppose a is 150% of b . What percent of a is b 3?设a 是b 的150%。
那么a 的百分之多少是3b ?A B C D E +3()50()66()150()200()45023. A box contains 28 red balls, 20 green balls, 19 yellow balls, 13 blue balls, 11 white balls, and 9 black balls. What is the minimum number of balls that must be drawnfrom the box without replacement to guarantee that at least 15 balls of a single color will be drawn?一个盒子中有28个红球,20个绿球,19个黄球,13个蓝球,11个白球和9个黑球。
为了保证至少取出15个单一颜色的球,在不允许放回重取的情况下,最少须从盒子中取出多少个球?A B C D E ()75()76()79()84()914. What is the greatest number of consecutive integers whose sum is 45? 最多可以有多少个连续整数,它们的总和是45?A B C D E ()9()25()45()90()1205. Two lines with slopes21and 2 intersect at (2,2). What is the area of the triangle enclosed by these two lines and the line +=x y 10?两条斜率分别为21和2的直线相交于(2, 2)。
2021年美国数学竞赛AMC12A 真题加答案解析
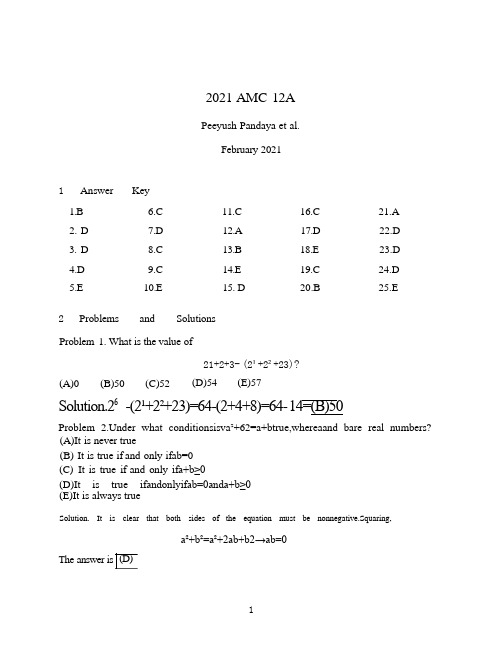
2021 AMC 12A Peeyush Pandaya et al.February 20211 Answer1.B2. D3. D4.D5.E Key6.C7.D8.C9.C10.E11.C12.A13.B14.E15. D16.C17.D18.E19.C20.B21.A22.D23.D24.D25.E2 Problems and SolutionsProblem 1. What is the value of21+2+3- (2¹+2²+23)?(A)0 (B)50 (C)52 (D)54 (E)57Solution.2⁶-(2¹+2²+23)=64-(2+4+8)=64-14=(B)50Problem 2.Under what conditionsisva²+62=a+btrue,whereaand bare real numbers?(A)It is never true(B) It is true if and only ifab=0(C) It is true if and only ifa+b≥0(D)It is true ifandonlyifab=0anda+b≥0(E)It is always trueSolution. It is clear that both sides of the equation must be nonnegative.Squaring,a²+b²=a²+2ab+b2→ab=0The answer is (D)Problem 3.The sum of two natural numbers is 17,402.One of the two numbers is divisible by 10.If the units digit of that number is erased, the other number is obtained. What is the difference of these two numbers?(A)10,272 (B)11,700 (C)13,362 (D)14,238 (E)15,426Solution. Let the first number mentioned be 10n;the second is n. Then10n+n=17,402,from which it follows thatProblem 4. Tom has a collection of 13 snakes,4 of which are purple and 5 of which are happy. He observes that●all of his happy snakes can add,·none of his purple snakes can subtract●all of his snakes that can't subtract also can't add.Which of these conclusions can be drawn about Tom's snakes?Solution. Together, the second and third conditions imply that none of Tom's purple snakes can add.Thus,(D) is correct: happy snakes are not purple.Problem 5.When a student multiplied the number 66 by the repeating decimal,where a and b are digits, he did not notice the notation and just multiplied 66 times 1.ab. Later he found that his answer is 0.5 less than the correct answer. What is the 2-digit integer gb? (A)15 (B)30 (C)45 (D)60 (E)75Solution. The student computed 66 ; the correct answer is 66 . Thus,Problem 6.A deck of cards has only red cards and black cards. The probability of a randomly chosen card being red is . When 4 black cards are added to the deck, the probability of choosing red becomes . How many cards were in the deck originally?(A)6 (B)9 (C) 12 (D)15 (E)18Solution. If the deck begins with x red cards and 3x cards in total, thenProblem 7.What is the least possible value of(ry- 1)²+(x+y)2 for real numbersa and y?Solution. We have(ry- 1)2+(x+y)2=(ry)2-2ry+1+x²+2ry+v²=x2v²+z²+y2+1= (r²+1)(y²+1),which achieves a minimum of (D)1 atx=y=0.D Do D ₁ D ₂D ₃ D ₁ D ₅D ₆ D ₇ D ₈ D, D1o Problem 8.A sequence of numbers isdefinedbyDo=0,D ₁=0,Dz=1,andDn=Dn- 1+Dn-3 forn≥3.What are the parities(evenness oroddness)of the triple of numbers(D2021,D2022,D2023), whereE denotes even and O denotes odd?Solution.0/10 01 D2+Do=1+0=1 D ₃+Di=1+0=1D ₄+Dz=1+1=0 Ds+D ₃=0+1=1D ₆+D4=1+1=0D-+Ds=0+0=0D ₈+D ₆=0+1=1Dg+D ₇=1+0=1We can see that the pattern repeats in cycles of length7.and as 2021=5 mod7,we have D2021= Ds,D2022=D6,D2023=D7→(C)(E,O,E) Problem 9.Which of the following is equivalent to(2+3)(2²+3²)(2⁴+34)(2⁸+3⁸)(216+316)(2³²+3³2)(264+364)?(A)3127+2127 (B)3127+2127+2.363+3.263 (C)3128-2128 (D)3128+2128 (E)5127Solution.(3-2)(2+3)(2²+3²)(2⁴+3⁴)(2⁸+3⁸)(216+316)(2³²+3³2)(264+364)=(3²-2²)(2²+3²)(2⁴+3⁴)(2⁸+3⁸)(216+316)(232+3³2)(264+364)=(3⁴-2⁴)(2⁸+3⁸)(216+316)(232+332)(264+364)=(C)3128-2129=(364-264)(264+364)Problem 10.Two right circular cones with vertices facing down as shown in the figure below contain the same amount of liquid. The radii of the tops of the liquid surfaces are 3cm and 6cm. Into each cone is dropped a spherical marble of radius lcm, which sinks to the bottom and is completely submerged without spilling any liquid. What is the ratio of the rise of the liquid level the narrow cone to the rise of the liquid level in the wide cone?(A)1:1 (B)47:43 (C) 2:1 (D)40:13 (E)4:1Solution. The two cones have equal volume, so the height of the first is times that of the second. Since the volumes increase by equal proportions, the heights increase by equal proportions. Thus, the ratio of the rise in liquid levels is (E)4:1Problem 11.A laser is placed at the point(3,5). The laser beam travels in a straight rry wants the beam to hit and bounce off the y-axis, then hit and bounce off the x-axis,then hit the point (7,5). What is the total distance the beam will travel along this path?(A)2√10 (B)5√2(C)10√2(D)15√2 (E)10√5Solution. Reflect about the y-axis then the z-axis. It is well-known that the image under the two reflections must be a straight line.The answer isv(3-(-7))²+(5-(-5)= (C) 10√2Problem 12.All the roots of polynomialz6- 10z ⁵+Az ⁴+Bz³+Cz²+Dz+16are positiveintegers, possibly repeated. What is the value of B?(A)-88 (B)-80 (C)-64 (D)-41 (E)-40Solution. By Vieta's,the sum of the 6 roots is 10 and the product is 16,hence they are all powers of 2. It is not hard to find that the only working unordered sextuple is(2,2,2,2,1,1). As(z-2)4=24-8z3+24z2-32z+16 and(z- 1)2=z2-2z+1.the z3 coefficient is -8.1+24 · (-2)+(-32) · 1= (B)-88Problem 13.Of the following complex numbers z, which has the property that z5 has the greatest real part?(A)-2 (B)-√3+i (C)-√2+√2i Solution. The magnitude of each complex number is the same, so it suffices to look at the argu- ment. The angles are π, ,and ,which after raising to the 5th power give π, and . We seek the angle that reaches farthest to the right(smallest argument),which is . Thus, our answer is (B)-√3+iProblem 14.What is the value of(A)21 (B)100logs3 (C)200log35 (D)2,200 (E)21,000Solution.And,Therefore, their product is210logs3.100log35=(E)21,000(D)- 1+√3i (E)2iProblem 15.A choir director must select a group of singers from among his 6 tenors and 8 basses. The only requirements are that the difference between the numbers of tenors and basses must be a multiple of 4,and the group must have at least one singer.Let be the number of groups that could be selected. What is the remainder when N is divided by 100?(A)47 (B)48 (C)83 (D)95 (E)96Solution. Suppose we mark down(1) the tenors that are in the group,and(2)the basses that aren't in the group. Then we necessarily mark down a number of people that is a multiple of 4. This is also sufficient; we mark down some people numbering a multiple of 4, then select the marked tenors and unmarked basses to form our choir. Clearly, we just mark down at least one person. The answer is thus(D)95Problem 16.In the following list of numbers, the integer n appears times in the list for l≤n≤200.1,2,2,3,3,3,4,4,4,4,.…,200,200,...200.What is the median of the numbers in this list?(A)100.5 (B)134 (C)142 (D)150.5 (E)167Solution.For general n, we have numbers. We want to approximate a such that is close to . Since the formula is a quadratic in n and we are halving this value,we can find that a is approximately .Plugging in n = 200,this is about 100√2,or 141.Of the answer choices, (C)142 is the closest, and indeed it is our answer.To verify, we can see that and ), so clearly 142 works.Problem 17.Trapezoid ABCD has ABICD,BC=CD=43,and AD1BD.Let O be the intersection of the diagonals AC and BD,and let P be the midpoint of BD.Given that OP=11, the length AD can be written in the form myn,where m and n are positive integers and n is not divisible by the square of any prime. What is m+n?(A)65 (B)132 (C)157 (D)194 (E)215Solution. Let M be the intersection of CPand AB.Since DCBMisakite,andCMIBD,we have MP1PB,and by considering the homothethy taking △MBD to △ABD with scale factor 2,we can see that M is the midpoint of AB.In particular,we haveSince AD 1BD,we have AD1DOandthusZADO=90°,andasCD=CB,wehaveCP1BD and ZCPD=2CPO=90°.Also,ZAOD=ZCOP,so △AOD~ △COP.Therefore,so DO=22.Thus,AD=√AB²-BD²=√86²-66²=4√ 190→m+n= (D)194The desired answer isProblem 18.Let f be afunction defined on the set of positive rational numbers with the property that f(a ·b)=f(a)+f(b)for all positive rational numbersa and b.Suppose that falso has the property that f(p)= pfor every prime numberp.For which of the following numbers zis f(x)<0?(A)整(B) (C) (D) (E) 51Solution. Note that f(a ·1)=f(a)+f(1)= f(1)=0,andIn particular, it follows by induction that f(p*)= kp for each k ∈Z.Thus,(A) f(2-5. 17)=-5·2+17=7(B) f(2-4. 11)=-4·2+11=3(C) f(3-2.7)=-2.3+7=1(D)f(2- 1.3- 1.7)=- 1.2+(- 1) ·3+7=2(E)f(52.11- 1)=2.5- 11=- 125The answer is (E)11Problem 19.How many solutions does the equation sinclosed interval [0,π]?(A)0 (B)1 (C) 2 (D) 3 (E)4Solution. Note on the interval , the left-hand side is negative while the right-hand side is positive.We thus restrict our attention to ]. The arguments cosx and sz are both between 0 and .ForsinA=cosBinA,B ∈[0,],we must have . This implies sinz+cosz=1,hence . There are (C) 2 solutions.Problem 20.Suppose that on a parabola with vertexV and focus F there exists a point Asuch that AF=20 and AV=21.What is the sum of all possible values of the length FV?(A)13 (B) 40 (C) (D)14 (E)Solution. Let the directrix be the x-axis,F=(0,2d),V=(0,d),A=(x,y),andB=(0,y)for some d>0.By the definition of a parabola,y=20.We compute x in two ways:x²=AF²-BF²=20²-|20-2d|2=AV²-BV²=21²-|20-d²Subtracting,O=20²-21²+(20-d)²-(20-2d)2=3d²-40d+41.The sum of all possible values ofProblem 21.The five solutions to the equation(z- 1)(z²+2z+4)(z²+4z+6)=0may be written in theformzk+ykiforl≤k≤5,where xk and yk are real.Let E be the unique ellipse that passes through the points(Ti,yi),(x2,32),(r3,Y3),(x4,y4)and(xs,ys).The eccentricity ofE can be written in the form ,where m and n are relatively prime positive integers. What is m+n?(Recall that the eccentrictiy of an ellipse E is the ratio,where 2a is the length of the major axis ofE and 2c is the distance between its two foci.)(A)7 (B)9 (C)11 (D)13 (E)15Solution. The roots of the polynomial arez=1,z=- 1±i√3,andz=-2±i√2,hence the five points onE are(1,0),( - 1,土√3),( - 2,±√2) .By symmetry through the x-axis, the ellipse is of the formE: a(x-r)²+by²=1.We then have the relationsa(1-r)²=1a(1+r)²+3b=1a(2+r)²+2b=1.Eliminating b from the latter two,1=3[a(2+r)²+2]-2[a(1+r)²+36]=a(r²+8r+10),henceit follows that and so the eccentricity is 1/√6. The requested sum is1+6= (A)7Problem 22.Suppose that the roots of the polynomialP(x)=x³+ax²+bx+care cos 2π,COS 47 and cos ,where angles are in radians. What is abc?(C) (D) (E)Solution. Recall1+e2m/7+ …+e12mi/7=0→e2mi/7+e4xi/7+e6mi/7=- 1/2,and in particular caNote are solutions to the equation cosb+cos28+cos30=- 1/2,so lettingx=co sθimplies thathas roots ,COS 4π7Thus, the polynomial in question is and the requested answer isRemark. Perhaps it is easier to motivate the solution as follows.Lett=e2mi/7andx=t+t- 1= 2cos2π/7.Thenx²-2=t²+t-2andx³-3x=t³+t-3.Moreover,t⁶+t⁵+ …+1=0impliest³+t²+t+t- 1+t-2+t-3=- 1,i.e.x+(x²-2)+(r³-3r) has root 2cos2π/7.It certainly seems logical that the Galois conjugates of 2cos would be 2cos and 2cos (especially given the phrasing of the problem),so simply replacex → to get the desired polynomial form.Let w = e2ix/7.Note thatLet these be r,s,t respectively. By Vieta's formulas, note that the desired quantity is(-rst)(rs+st+tr)(-r-s-t)=(r+s+t)(rs+st+tr)(rst).Note that 1+w+ …+w⁶=0.We haveThen,FinallyMultiplying yields the answer of (D)1 32Solution Manual 2021AMC12AProblem 23.Frieda the frog begins a sequence of hops on a 3×3 grid of squares,moving one square on each hop and choosing at random the direction of each hop up,down, left,or right. She does not hop diagonally. When the direction of a hop would take Frieda off the grid,she ”wraps around”and jumps to the opposite edge.For example if Frieda begins in the center square and makes two hops ”up”,the first hop would place her in the top row middle square, and the second hop would cause Frieda to jump to the opposite edge,landing in the bottom row middle square.Suppose Frieda starts from the center square, makes at most four hops at random,and stops hopping if she lands on a corner square. What is the probability that she reaches a corner square on one of the four hops?(A)G (B) (D)强(E)8Solution. We complementary count,and determine the probability we never reach a corner square. Denote by A the center,and B a square adjacent to the center. Then the first hop lands on B.● If the second hop lands on A(with probability ,then the third hop lands on B always,andthere is a chance the fourth hop lands on a non-corner square. The probability in this case is● If the second hop lands on B, then there is a chance the third hop lands on A,and achance the third hop lands on B.In the former subcase, the fourth hop always lands on a non-corner square, and in the latter subcase, there is a chance the fourth hop lands on a non-corner square. The probability in this case isThe requested probability isProblem 24.SemicircleT has diameter AB of length14.Circle Ωlies tangent to AB at a point P and intersectsI at pointsQandR.IfQR=3√3and ZQPR=60°,then the area of △PQR is ,where a and c are relatively prime positive integers, and b is a positive integer not divisible by the square of any prime. What isa+b+c?(A)110 (B)114 (C)118 (D)122 (E)126Solution. First, by Extended Law of Sines,we have that the radius of Q is .Let M be the midpoint of QR,O be the center ofT,and X be the center of 2.Since △OQR is isosceles, OM is perpendicular to QR. Thus we have that X lies on OM since it is the circumcenter of △puting lengths, we hav thagorean theorem on △ORM andfrom isosceles triangle XQR. Thus and OP=4 from Pythagorean theorem on △OXP.To find [PQR],we will find the height from P to QR.Let the foot of the perpendicular fromX to the P-altitude be D. Since PDⅡOM,we know that △XDP~△OPX.This means that . Now note that the bottom portion of the P-altitude after subtractingPD is equal to XM,so the height of the triangle is . The area is simply2021 AMC 12A11 Solution ManualProblem 25.Let d(n)denote the number of positive integers that divide n,including l and n. For example,d(1)=1,d(2)=2,and d(12)=6.(This function is known as the divisor function.) LetThere is a unique positive integer N such that f(N)> f(n)for all positive integers n≠N.What is the sum of the digits of N?(A)5 (B)6 (C)7 (D)8 (E) 9Solution. Letn=II;p',wherepi=2,Pz=3,etc.are the primes in increasing order and e; are nonnegative integers. ThenIt is equivalent to maximize Thus, it remains to find the optimal e; for each i. We go term-by-term, noting that it is only necessary to check until the expression first decreases, as exponentials increase more quickly than polynomials.ei 0 1 2 3 4 0 1 2 3 0 1 2 0 1 2 ((ez+1)3)/p⁸1³/20=1 23/2¹=433/2²=6.25 4³/2³=85³/2⁴<8 13/3⁰=1 23/31≈2.67 3³/32=3 43/3³<3 13/5⁰=1 2³/5¹=1.6 3³/5²=1.08 13/70=1 23/71≈1.14 3³/7²<1Note that we do not need to check p≥11,ase;=1yields which is suboptimal.Thus,the answer isN=23.32.5.7=2520,which has a Remark:It is sufficient to stop at p=3,for 3²|Nleaves N among the answer choices.digit sum of (E)9only one possibility for the digit sum ofi 1 1 1 1 1 2 2 2 2 3 3 3 4 4 4。
美国中学数学竞赛(AMC12-12级)(附答案)

2004 AMC12 试题1. 爱丽每小时的工资为美金20元,其中的1.45%要缴地方税,试问爱丽每小时的工资中要付地方税美金多少分?(美金1元二美金100分)(A) 0.0029 (B) 0.029 (C) 0.29 (D) 2.9 (E) 292. 于AMC2的测验中,每答对一题可得6分,答错可得0分,未作答可得2.5分.假设凯琳在25题中有8题未作答,她至少要答对几题,总分才会不低于100 分?(A) 11(B) 13(C) 14(D) 16(E) 173.滿足x 2y100的正整数序对(x, y)有多少組?(A) 33(B) 49(C) 50(D) 99(E) 1004.白婆婆有6个女儿、没有儿子,有些女儿也恰有6个女儿,其他的女儿没有孩子. 白婆婆有女儿及外孙女共30位,没有外曾孙女. 试问白婆婆的女儿及外孙女中有多少位没有女儿?(A) 22 (B) 23 (C) 24 (D) 25 (E) 265.直线y mx(A) mb 1 (B) 1 mb 0 (C) mb 0 (D) 0 mb 1 (E) mb 16.设u 2 20042005, V 20042005, W 2003 20042004, X 2 20042004Y 20042004, Z 20042003.試问以下何者值最大?(A) U V (B) V W (C) W X (D) X Y (E) Y Z7. 一种代币的游戏,其规那么如下:每回持有最多代币者须分给其他每一位参与游戏者一枚代币,并放一枚代币於回收桶中;当有一位游戏参与者没有代币时,那么游戏结束.假设A、B C三人玩此游戏,在游戏开始时分别持有15、14及13枚代币.试问游戏从开始到结束,共进行了多少回?(A) 36 (B) 37 (C) 38 (D) 39 (E) 409. 有一个将花生酱装在圆桶状瓶子内出售的公司 .市场研究建议瓶子较粗时可增加销售量.假设瓶子的直径增加25%,而体积仍维持不变,那么 瓶子的高度应减少百分之多少?(A) 10(B) 25(C) 36(D) 50(E) 6010. 有49个连续整数,它的和为75,則它们排在最中间的数为何?(A) 7(B) 72(C) 73(D) 74(E) 7511. 某国的硬币中有1分、5分、10分及25分四种,在保拉的皮包 內硬币的平均值为20分.假设再增加一枚25分的硬币,平均值則增为 21分.試问她的皮包內有多少枚10分的硬币?(A) 0(B) 1(C) 2(D) 3(E) 412. 设A (0, 9) , B (0,12).点A 、B 在直线y x 上, 且AA 与BB 交于点 C (2,8).試问AB的长度是多少?(A) 2(B) 2 2(C) 3(D) 22(E) 3“13. 以S 表示坐标平面上所有的点(a,b)所形成的集合,其中a,b 等于1, 0,或1.試问有多少条相异的直线至少通过集合 S 中的两个点?(A) 8(B) 20(C) 24(D) 27(E) 3614. 三个实数的数列形成一个等差数列,首项是9.假设将第二项加2、第 三项加20可使得这三个数变为等比数列,那么这个等比数列中第三项 最小可能是多少?(A) 1(B) 4(C) 36(D) 49(E) 8115.小美与小雯在一个圆形的跑道上向相反的方向跑 ,开始两人分别从8.如下图EAB 及 ABC 为直角,厢 4 , BC 6 , AE 8, AC 与BE 交于D 点.試问 差为多少?(A) 2(B) 4(C) 5 (D) 8 (E) 9ADE 与BDC 面积之圆形跑道直径的两端起跑.小美跑了 100公尺时她们第一次相遇;在 第一次相遇后小雯跑了 150公尺时她们第二次相遇.假设她们跑的速 度都分别维持固定不变,试问此圆形跑道的长度是多少公尺?(A) 250(B) 300(C) 350(D) 400(E) 50016.使函数 log 2004{log 2003{log 2002{log 2001 X}}}有定义的集合为 之值是多少?(A) 0(B) 20012002(C) 20022003(D) 20032004{xx c}.試问 c(E) 20012°°丹317.函数f 满足以下性质: (i) f(1) 1,且(ii)对任意的正整数n ,f(2n) n f(n).试问f(2100)之值为何?(A) 1(B) 299(C) 2100(D) 24950 18.如下图,ABCD 是边长2的正方形.在正方形 的内部作一个以AB 为直径的半圆,且自C 点引 此半圆的切线交AD 边于E 点•试问CE 的长度是 多少?(E) 5 ■.5(E) 29999(B) 5 (C) 6 (D)?19.如下图,A B C 三圆彼此外切且均内切于圆等,圆A 的半径1且通过圆D 的圆心.试问圆B 的半径是多少?D.B 、C 两圆相(A)3(B)于(C) 土 (D) |20.从0与1之间的数,随机独立取出两实数a 与b,并将a, b 之和记 作c.分别以A, B, C 表示最接近a, b, c 的整数.试问 的机率为何?(A) 1(B) !(C) 1(D) 2(E) 443 23421.假设n 02nCOS5,那么Cos2之值是多少?(A) 15 (B) I (C) ±55(D) ?(E)上5522.三个半径为1的球彼此外切且放置在一水平面上,一个半径为2 的大球放在它们的上面 .试问大球的最高点至平面的距离是多 少?C 2004 0,且 P (x)=0 有 2004个复数根 z a k b k i , 20042004b k 为实数,a ib i 0,且a kb k .k 1k 1试问以下哪一项可能不是 0 ?2004(A) C 0(B)C2003(C) b z b s L b s 004 (D)a kk 124. 设A 、B 为平面上的两点,其中AB 1.令S 为平面上所有半径是1且 能盖住线段AB 之圆的并集,則S 的面积是多少?(A) 2.3(B) -(C) 33(D)卫 3(E) 4 2 33 2325. 对每一个整数n 4,令a n 表示n 进位的循环小数0.133n .把乘积a 4a 5L a 99写成—的形式,其中m P 为正整数,且p 尽可能小.试问mp!之值是多少?(A) 98(B) 101(C) 132(D) 798(E) 962Q屈o J123 52(A) 3(B) 3 -(C) 3 -(D)2349(E) 3 2、223.多项式P(x) GX C 0 ,的系数都是实数, 1 k 2004,其中 a k ,2004(E)C kk 12004C 2004X 2003C 2003XL答案:1 ( E )2 ( C )3 ( B )4 ( E )5 ( B )6 ( A )7 ( B )8 ( B )9 ( C )10 ( C ) 11 ( A )12 ( B )13 ( B )14 ( A )15 ( C ) 16 ( B )17 ( D )18 ( D )19 ( D )20( E ) 21 ( D )22 ( B )23 ( E )24 ( C )25 ( E )。
AMC美国数学竞赛简介

AMC美国数学竞赛简介American Mathematics Competition又称为American High School Mathematics Examinatio n美国中学数学科考试(AHSME),是由美国数学协会(Mathematics Association of America)于1950年成立,目前总部设于美国内布拉斯加大学林肯校区(University Of Nebraska-Lincoln),是美国数学协会(Mathematics Association of America)的直属机构。
AMC的三种级别AMC8 简介 AMC8是针对初中一年级、初中二年级学生的数学测验,25题选择题、考试时间40分钟。
其测验目的是为了增进学生对数学习题解答的能力。
这项测验提供了一些中学程度的数学概念的教学与评量;其题目范围不仅是由易而难,而且还涵盖了较广泛的数学实际应用。
其中的一些题目颇具挑战性,程度高于一般的中学数学。
因此,不失为一个良好的数学经验。
AMC8的测验允许使用计算器(工程用计算器除外);此外,其成绩表现不错的学生也将被邀请参加AMC10 测验。
AMC8有一个特别的目的:是希望使这些题目能利用在各中学数学课程的实际教学上。
AMC8测验可激发学生增加对数学理解能力的潜能。
除了AMC8之外,还有其接下来的各项测验都能刺激学生产生对于数学课程的兴趣。
另外,AMC8尚可增进且鼓舞学生对于数学学习抱持着更积极的态度,并引起学生对数学的喜好。
对学习者而言,AMC8是有助于对数学观念的理解和进步。
但重要的是,必须抱持着积极的学了这样的一个机会。
我们竭诚欢迎初中一年级及初中二年级的学生参加AMC8测验;无论你身在何处,只要你是初中二年级或初中二年级以下的学生就能有资格参加AMC8的测验。
AMC10 简介AMC10是针对高中一年级及初中三年级学生的数学测验,25题选择题、考试时间75分钟;包含演算概念理解的数学题型。
2021年美国数学竞赛AMC-12A 真题加答案解析(英文版)
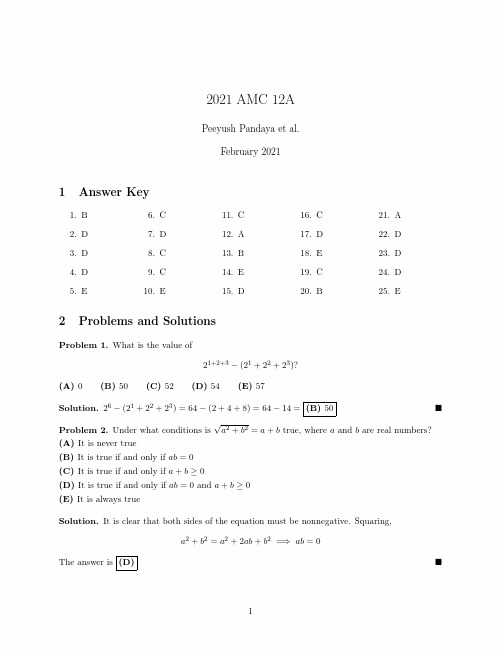
(A) 15 (B) 30 (C) 45 (D) 60 (E) 75
⇣
⌘
⇣
⌘
Solution. The student computed 66
1
+
ab 100
; the correct answer is 66
1
+
ab 99
. Thus,
✓
◆
66 · (a b) ·
1 99
1 100
= 0.5 =) a b = (E) 75
⌅
p Problem 2. Under what conditions is a2 + b2 = a + b true, where a and b are real numbers?
(A) It is never true
(B) It is true if and only if ab = 0
(C) It is true if and only if a + b 0
• all of his happy snakes can add, • none of his purple snakes can subtract
• all of his snakes that can’t subtract also can’tonclusions can be drawn about Tom’s snakes?
(A) 10, 272 (B) 11, 700 (C) 13, 362 (D) 14, 238 (E) 15, 426
Solution. Let the first number mentioned be 10n; the second is n. Then 10n + n = 17, 402, from
1998年美国数学竞赛-AMC_12
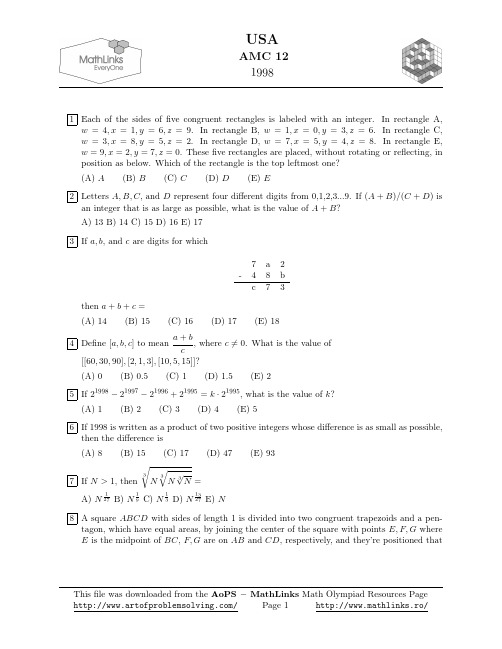
19981Each of the sides of five congruent rectangles is labeled with an integer.Inrectangle A,w =4,x =1,y =6,z =9.In rectangle B,w =1,x =0,y =3,z =6.Inrectangle C,w =3,x =8,y =5,z =2.In rectangle D,w =7,x =5,y =4,z =8.Inrectangle E,w =9,x =2,y =7,z =0.These five rectangles are placed,without rotating orreflecting,inposition as below.Which of the rectangle is the top leftmost one?(A)A (B)B (C)C (D)D (E)E 2Letters A,B,C,and D represent four different digits from 0,1,2,3...9.If (A +B )/(C +D )is an integer that is as large as possible,what is the value of A +B ?A)13B)14C)15D)16E)173If a,b,and c are digits for which7a 2-48b c 73then a +b +c =(A)14(B)15(C)16(D)17(E)184Define [a,b,c ]to mean a +b c,where c =0.What is the value of [[60,30,90],[2,1,3],[10,5,15]]?(A)0(B)0.5(C)1(D)1.5(E)25If 21998−21997−21996+21995=k ·21995,what is the value of k ?(A)1(B)2(C)3(D)4(E)56If 1998is written as a product of two positive integers whose difference is as small as possible,then the difference is(A)8(B)15(C)17(D)47(E)937If N >1,then 3 N 3 N 3√N =A)N 127B)N 19C)N 13D)N 1327E)N 8A square ABCD with sides of length 1is divided into two congruent trapezoids and a pen-tagon,which have equal areas,by joining the center of the square with points E,F,G where E is the midpoint of BC ,F,G are on AB and CD ,respectively,and they’re positioned that This file was downloaded from the AoPS −MathLinks Math Olympiad Resources PagePage 1http://www.mathlinks.ro/1998AF <F B,DG <GC and F is the directly opposite of G .If F B =x ,the length of the longer parallel side of each trapezoid,find the value of x .(A)35(B)23(C)34(D)56(E)789A speaker talked for sixty minutes to a full auditorium.Twenty percent of the audience heard the entire talk and ten percent slept through the entire talk.Half of the remainder heard one third of the talk and the other half heard two thirds of the talk.What was the average number of minutes of the talk heard by members of the audience?(A)24(B)27(C)30(D)33(E)3610A large square is divided into a small square surrounded by four congruent rectangles asshown.The perimeter of each of the congruent rectangles is 14.What is the area of the large square?[img]/Forum/album p ic.php ?pic i d =460[/img ](A)49(B)64(C)100(D)121(E)19611Let R be a rectangle.How many circles in the plane of R have a diameter both of whose endpointsare vertices of R?A.1B.2C.4D.5E.612How many different prime numbers are factors of N iflog 2(log 3(log 5(log 7N )))=11?(A)1(B)2(C)3(D)4(E)713Walter rolls four standard six-sided dice and finds that the product of the numbers on the upperface is 144.Which of the following could NOT be on the sum of the upper four faces?(A)14(B)15(C)16(D)17(E)1814A parabola has vertex at (4,-5)and has two x -intercepts,one positive and one negative.If thisparabola is the graph of y =ax 2+bx +c ,which of a,b,and c must be positive?(A)Only a (B)Only b (C)Only c (D)Only a and b (E)None 15A regular hexagon and an equilateral triangle have equal areas.What is the ratio of the length ofa side of the triangle to the length of a side of the hexagon?A.√3B.2C.√6D.3E.6199816The figure shown is the union of a circle and two semicircles of diameters of a and b ,all of whosecenters are collinear.The ratio of the area of the shaded region to that of the unshaded region is (A) a b (B)a b (C)a 2b (D)a +b 2b (E)a 2+2ab b +2ab 17Let f (x )be a function with the two properties:(a)for any two real numbers x and y ,f (x +y )=x +f (y ),and (b)f (0)=2What is the value of f (1998)?A.0B.2C.1996D.1998E.200018A right circular cone of volume A ,a right circular cylinder of volume M ,and a sphere of volumeC all have the same radius,and the common height of the cone and the cylinder is equal to the diameter of the sphere.Then(A)A −M +C =0(B)A +M =C (C)2A =M +C (D)A 2−M 2+C 2=0(E)2A +2M =3C 19How many triangles have area 10and vertices at (-5,0),(5,0),and (5cos θ,5sin θ)for some angleθ?A.0B.2C.4D.6E.820Three cards,each with a positive integer written on it,are lying face-down on a table.Casey,Stacy,and Tracy are told that(a)the numbers are all different,(b)they sum to 13,and (c)they are in increasing order,left to rightFirst,Casey looks at the number on the leftmost card and says,”I don’t have enough information to determine the other two numbers.”Then Tracy looks at the number on the rightmost card and says,”I don’t have enough information to determine the other two numbers.”Finally,Stacy looks at the number on the middle card and says,”I don’t have enough information to determine the other two numbers.”Assume that each perosn knows that the other two reason perfectly and hears their comments.What number is on the middle card?A.2B.3C.4D.5E.There is not enough information to determine the number.21In an h -meter race,Sunny is exactly d meters ahead of Windy when Sunny finishes the race.Thenext time they race,Sunny sportingly starts d meters behind Windy,who is at the starting line.Both runners run at the same constant speed as they did in the first race.How many meters ahead is Sunny when Sunny finishes the second race?(A)d h (B)0(C)d 2h (D)h 2d (E)d 2h −d199822What is the value of the expression 1log 2100!+1log 3100!+1log 4100!+···+1log 100100!!(A)0.01(B)0.1(C)1(D)2(E)1023The graphs of x 2+y 2=4+12x +6y and x 2+y 2=k +4x +12y intersect when k satisfies a ≤k ≤b ,and for no other values of k .Find b −a .A.5B.68C.104D.140E.14424Call a 7-digit telephone number d 1d 2d 3−d 4d 5d 6d 7MEMORABLE if the prefix sequence d 1d 2d 3is exactly the same as either of the sequences d 4d 5d 6or d 5d 6d 7(possibly both).Assuming that each d i can be any of the ten decimal digits 0,1,2,...9,the number of different memorable telephone numbers is(A)19810(B)19910(C)19990(D)20000(E)2010025A piece of graph paper is folded once so that (0,2)is matched with (4,0)and (7,3)is matched with(m,n ).Find m +n .A.6.7B.6.8C.6.9D.7.0E.8.026In quadrilateral ABCD ,it is given that ∠A =120◦,angles B and D are right angles,AB =13,and AD =46.Then AC =(A)60(B)62(C)64(D)65(E)7227A 9x 9x 9cube is composed of twenty-seven 3x 3x 3cubes.The big cube is ’tunneled’as follows:First,the six 3x 3x 3cubes which make up the center of each face as well as the center of 3x 3x 3cube are removed.Second,each of the twenty remaining 3x 3x 3cubes is diminished in the same way.That is,the central facial unit cubes as well as each center cube are removed.The surface area of the final figure isA.384B.729C.864D.1024E.105628In triangle ABC ,angle C is a right angle and CB >CA .Point D is located on BC so that angleCAD is twice angle DAB .If AC/AD =2/3,then CD/BD =m/n ,where m and n are relatively prime positive integers.Find m +n .A.10B.14C.18D.22E.2629A point (x,y)in the plane is called a lattice point if both x and y are integers.The area of thelargest square that contains exactly three lattice points in its interior is closest toA.4.0B.4.2C.4.5D.5.0E.5.6199830For each positive integer n ,let:a n =(n +9)!(n −1)!If k is the smallest integer with the rightmost nonzero integer a k is odd.The rightmost nonzero digit of a k is A)1B)3C)5D)7E)9。
amc12美国数学竞赛

amc12美国数学竞赛
AMC 12是美国数学竞赛(American Mathematics Competition)的简称,是由美国数学协会(American Mathematical Society)举办的年度数学竞赛。
它是美国最受欢迎的高中数学竞赛之一,每年有数千名学生参加。
AMC 12的考试内容包括高中数学的常规知识,如代数、几何、解析几何、排列组合、概率和统计等。
考试形式为选择题和填空题,每年的考试都是在12月的第二个星期二进行。
AMC 12的成绩被用于选择参加更高级别的数学竞赛,如美国大学生数学竞赛(AIME)和美国国家队数学竞赛(USAMO)等。
参加并取得优异成绩的学生还有机会获得大学奖学金和其他荣誉。
2020年美国数学竞赛(AMC12B)的试题与解答

2020年美国数学竞赛(AMC12B)的试题与解答华南师范大学数学科学学院(510631)李湖南1.下列表达式√1+√1+3+√1+3+5+√1+3+5+7的最简值是多少?(A)5(B)4+√7+√10(C)10(D)15(E)4+3√3+2√5+√7解直接计算,原式=1+2+3+4=10,故(C)正确.2.下列表达式1002−72702−112·(70−11)(70+11)(100−7)(100+7)的值是多少?(A)1(B)99519950(C)47804779(D)108107(E)8180解由平方差公式可知,原式=1,故(A)正确.3.已知w 与x 之比为4:3,y 与z 之比为3:2,z 与x之比为1:6.则w 与y 之比是多少?(A)4:3(B)3:2(C)8:3(D)4:1(E)16:3解化简即得w y =w x ·x z ·z y =43·61·23=163,故(E)正确.4.一个直角三角形的两个锐角分别是a ◦和b ◦,其中a >b 且a 和b 均为素数.则b 最小可能的值是多少?(A)2(B)3(C)5(D)7(E)11解问题转化为求a +b =90的最小素数解,可解得a =83,b =7,故(D)正确.5.球队A 和B 正在打篮球联赛,每场比赛都要决出胜负.球队A 赢了23的比赛,球队B 赢了58的比赛,而且球队B 比球队A 多赢了7场比赛也多输了7场比赛.则球队A 打了多少场比赛?(A)21(B)27(C)42(D)48(E)63解设球队A 和B 分别打了x ,y 场比赛,依题意有: 58y −23x =7,38y −13x =7,解方程组得x =42,y =56.故(C)正确.6.对于所有整数n 9,(n +2)!−(n +1)!n !的值是以下哪个?(A)4的倍数(B)10的倍数(C)一个素数(D)一个平方数(E)一个立方数解(n +2)!−(n +1)!n !=(n +1)·(n +1)!n !=(n +1)2,它是一个平方数,故(D)正确.7.xy -坐标平面上的两条非水平、非竖直的直线相交于点O ,且夹角为45◦.一条直线的斜率是另一条直线斜率的6倍.则这两条直线斜率乘积的最大值是多少?(A)16(B)23(C)32(D)3(E)6解设两条直线的方程分别为y =kx,y =6kx ,倾斜角分别为α,β,依题意可得β=α±45◦.于是6k =tan β=tan (α±45◦)=tan α±tan 45◦1∓tan α·tan 45◦=k ±11∓k,化简得±6k 2−5k ±1=0,解得k =±12,±13.所求的最大值为(k ·6k )max =32.故(C)正确.由假设x 2>1,x 21±2x 1√3−3x 21+(3−3x 21)>4,4x 21(3−3x 21)>(1+2x 21)2,16x 41−8x 21+1<0,(4x 21−1)2<0.该不等式在实数范围内不成立,由矛盾可知假设错误,原命题得证.三、总结本例中解法一的求导分析法比较常规,学生容易想到,但运算过程冗长,思路固定化,不利于培养学生的发散性思维;解法二对学生思维的灵活性要求较高,可以类比一元二次方程,逐步深入,适当引导学生探究一元三次方程的相关解法;解法三利用反证法,正难则反,即间接证明的思路帮学生打破僵化思维,开拓解题视野.高中各章节数学知识之间存在着内在联系,呈现出很强的层次性和系统性,如函数与方程、零点与实根,直接证法和间接证法.为帮助学生将这些看似无关的知识整合起来,教师在教学活动中应当坚持问题驱动原则,提炼数学思想方法,有意识地引导学生从不同角度剖析问题,进行多解尝试.此外,教师在教学中应当“道而弗牵,强而弗抑,开而弗达”,这样学生有自主思考的空间才能举一反三,事半功倍.8.有多少个整数对(x,y)满足方程x2020+y2=2y?(A)1(B)2(C)3(D)4(E)无穷多解原方程可化为x2020+(y−1)2=1,容易解得x=1,y=1,x=−1,y=1,x=0,y=0,x=0,y=2,故满足方程的解共有4对,(D)正确.9.如图示,一个半径为4英寸的圆的四分之三连同它的内部正好可以卷成一个圆锥的侧面,如果将它的两条半径无缝连接.则这个圆锥的体积是多少立方英寸?(A)3π√5(B)4π√3(C)3π√7(D)6π√3(E)6π√7解设这个圆锥的底面半径和高分别为r和h,则有2πr=34·2π·4,r2+h2=42,解得r=3,h=√7.于是该圆锥的体积为V=13πr2h=3π√7,故(C)正确.10.在单位正方形ABCD中,内接圆ω交CD于点M,AM交ω于另一点P.则AP是多少?(A)√512(B)√510(C)√59(D)√58(E)2√515解如右图示,AD=1,M,N均为圆与正方形的切点,则M,N分别为CD,AD的中点,即AN=DM=12.从而AM=√AD2+DM2=√52,由切割线定理AN2=AP·AM,可得AP=AN2AM=√510.故(B)正确.11.如图所示,一个边长为2的正六边形的内部有6个半圆,这些半圆的直径恰好是该六边形的6条边.则图中阴影部分—–六边形内部半圆以外的面积是多少?(A)6√3−3π(B)9√32−2π(C)3√32−π3(D)3√3−π(E)9√32−π解S六边形=6·S半圆+S阴影−6·S树叶,其中树叶为两个半圆的交集部分.而S六边形=6×12·2·√3=6√3,S半圆=12×π·12=π2,树叶恰好是半圆内一个60◦的扇形去掉一个正三角形的部分的两倍,S树叶=2×(16π−12·√32)=π3−√32,于是有S阴影=S六边形−6·S半圆+6·S树叶=6√3−6·π2+6·(π3−√32)=3√3−π.故(D)正确.12.设AB是半径为5√2的圆中的一条直径,CD是圆内的一条弦,交AB于点E,使得BE=2√5,∠AEC=45◦.则CE2+DE2是多少?(A)96(B)98(C)44√5(D)70√2(E)100解如图示,过圆心O作OF⊥CD于点F,则F是CD的中点,且∆OEF是等腰直角三角形.由题意可知OB=5√2,BE=2√5,从而OE=5√2−2√5,EF=√22OE=5−√10,于是CE=CF+EF=DE+2EF=DE+10−2√10.另外,根据相交弦定理有CE·DE=AE·BE=(10√2−2√5)·2√5=20(√10−1).从而CE2+DE2=(DE+10−2√10)2+DE2=2[DE2+(10−2√10)DE]+140−40√10=2DE·CE+140−40√10=100故(E)正确.13.以下哪个等于√log26+log36?(A)1(B)√log56(C)2(D)√log23+√log32(E)√log26+√log36解原式=√log22·3+log32·3=√log23+2+log32=√(√log23+√log32)2=√log23+√log32故(D)正确.14.贝拉和珍在实数轴的闭区间[0,n]上玩如下游戏,其中给定整数n>4.他们轮流玩,贝拉先开始.最开始,贝拉在[0,n]上选择一个任意实数.然后,接下来轮到的选手要选择一个与他们之前选择的所有实数距离都要大1个单位的实数.无法选择的选手就算输.使用最优策略,哪位选手会赢得这个游戏?(A)贝拉得胜(B)珍得胜(C)当且仅当n是奇数时贝拉得胜(D)当且仅当n是奇数是珍得胜(E)当且仅当n>8时珍得胜解贝拉将赢得这个游戏.因为最开始贝拉选择n2,将区间分成两个对称的小区间[0,n2]和[n2,n],接下来无论珍在哪个区间中选择什么数,贝拉都将选择另一个区间中关于n2中心对称的那个数.只要珍能够选择数,贝拉就可以继续游戏.到最后,无法选择的一定是珍,即珍必败,故(A)正确.15.10个人等距地围坐在一个圆周上,每个人都只认识其余9人中的3人:与他或她相邻的2人,以及圆周上相对的那个人.问有多少种方式把这10个人分成5对,使得每对的两个人都互相认识?(A)11(B)12(C)13(D)14(E)15解如图示,分别给这10个人编号为1,2,3,…,10,互相认识的人用线段或弧线连接,先考虑1号,分如下三种情况:(1)1与2配对:若3与4配对,则有{5,6},{7,8},{9, 10},或{5,10},{6,7},{8,9},共2种方式;若3与8配对,则7必与6配对,有{4,5},{9,10},或{4,9},{5,10},也是2种方式.(2)1与10配对:同理可得4种方式;(3)1与6配对:若2与3配对,则7必与8配对,有{4, 5},{9,10},或{4,9},{5,10},共2种方式;若2与7配对,则有{3,4},{5,10},{8,9},或{3,8},{4,5},{9,10},或{3,8}, {4,9},{5,10},共3种方式.综上可得,符合条件的分法共有13种.故(C)正确.16.缸里有1个红球和1个蓝球,旁边另有一盒子红球和蓝球.乔治进行如下操作四次:首先他从缸里随机拿起一个球,然后他从盒子里拿起一个相同颜色的球,接着把两个球放回缸里.四次操作之后,缸里有6个球.则缸里有两种颜色各3个球的概率是多少?(A)16(B)15(C)14(D)13(E)12解四次操作之后,缸里可能有1,2,3,4,5个红球.根据对称性,剩1个红球和1个蓝球即5个红球的概率是一样的, 2个红球和4个红球的概率是一样的.剩1个红球,也即乔治每次拿起的都是蓝球,从而P(1个红球)=12·23·34·45=15;剩2个红球,也即乔治四次操作中只拿起一次红球,其中第1,2,3,4次均有可能,从而P(2个红球)=P(只第1次拿红球)+P(第2次)+P(第3次)+P(第4次)=12·13·24·35+12·13·24·35+12·23·14·35+12·23·34·15=15因此,P(3个红球)=1−2×P(1个红球)−2×P(2个红球)=15.故(B)正确.17.有多少个形式为x5+ax4+bx3+cx2+dx+2020,其中a,b,c,d是实数的多项式具备以下性质:只要r是一个根,−1+i√32·r也是?(注意i=√−1.)(A)0(B)1(C)2(D)3(E)4解令−1+i√32=ω,则有ω2+ω+1=0,ω3=1.设所求多项式为f(x),由于复数根总是成对出现,从而f(x)必有实根r,依题意ωr,ω2r也是f(x)的根.根据代数基本定理,f(x)在复数域内有5个根,故其余两根必是重根.分两种情况:(1)f(x)的5个根为r,r,r,ωr,ω2r,则f(x)=(x−r)3(x−ωr)(x−ω2r),根与系数的关系式为−a=3r+rω+rω2,b=4r2+3r2ω+3r2ω2,−c=4r3+3r3ω+3r3ω2,d=3r4+r4ω+r4ω2,−2020=r5,解得r=−5√2020,a=−2r,b=r2,c=−r3,d=2r4;(2)f(x)的5个根为r,ωr,ωr,ω2r,ω2r,则f(x)=(x−r)(x−ωr)2(x−ω2r)2,根与系数的关系式为−a=r+2rω+2rω2,b=4r2+3r2ω+3r2ω2,−c=4r3+3r3ω+3r3ω2,d=r4+2r4ω+2r4ω2,−2020=r5,解得r=−5√2020,a=r,b=r2,c=−r3,d=−r4;综上可得,符合条件的多项式只有2个,故(C)正确.18.在正方形ABCD中,点E,H分别在边AB和DA上,AE=AH,点F,G分别在边BC和CD上,点I,J在边EH上,使得F I⊥EH,GJ⊥EH.如下图所示,三角形AEH,四边形BF IE,DHJG和五边形F CGJI的面积均为1.则F I2是多少?(A)73(B)8−4√2(C)1+√2(D)74√2(E)2√2解由题意知,S ABCD=4,AE=√2,从而AB=2,BE=2−√2.作四边形BF IE关于BF的对称图形BF KL,分别延长IE和KL且相交于点M,连接BM,则可得四边形F KMI是一个正方形,如右图示.于是F I2=S F KMI=2·(S BF IE+S∆BEM)=2·[1+12×(2−√2)2]=8−4√2,故(B)正确.19.坐标平面中的正方形ABCD顶点分别为A(1,1),B(−1,1),C(−1,−1),D(1,−1).考虑以下四种变换:L:绕原点做90◦的逆时针旋转;R:绕原点做90◦的顺时针旋转;H:关于x轴的反射;V:关于y轴的反射.每一种变换都将这个正方形映回自身,但顶点的位置会发生变化.比如,施行R和V会将点A由(1,1)映到(−1,−1),将点B由(−1,1)映回自身.从集合{L,R,H,V}中选择20个变换,有多少种顺序方式可以将四个顶点均映回自身?(比如,R,R,V,H就是一种4个变换的顺序方式,它将四个顶点均映回自身.)(A)237(B)3·236(C)238(D)3·237(E)239解我们记正方形ABCD经过变换后得到的正方形分别在第一、二、三、四象限的顶点顺序.例如:变换L将ABCD映成DABC,LL将ABCD映成CDAB.经过一对变换之后,结果只可能为如下四种:(1)ABCD映成ABCD:恒等变换,通过4种方式LR,RL,HH,V V可得;(2)ABCD映成CDAB:相当于中心对称变换,通过4种方式LL,RR,HV,V H可得;(3)ABCD映成ADCB:相当于交换B,D两点,通过4种方式LV,RH,HL,V R可得;(4)ABCD映成CBAD:相当于交换A,C两点,通过4种方式LH,RV,HR,V L可得.因此,经过前面18个任意变换之后,其结果也只可能是ABCD,CDAB,ADCB,CBAD中的一种,且是等概率的.此时,只需要再经过一对变换即可映回ABCD,且均有4种方式.故将ABCD映回自身的方式共有418×4=238种,(C)正确.20.两个相同尺寸的不同立方体将被涂色,每个面的颜色被独立、随机地涂成黑色或白色.则涂色后两个立方体调整后在外观上完全相同的概率是多少?(A)964(B)2892048(C)73512(D)1471024(E)5894096解两个立方体共有12个面,从而有212种涂色的方式,分情况讨论:(1)两个立方体均为全黑色,只有1种方式;(2)两个立方体均为5黑1白,有6×6=36种方式;(3)两个立方体均为4黑2白:如果两个白色面相对,有3×3=9种方式;如果两个白色面相邻,有12×12=144种方式;(4)两个立方体均为3黑3白:如果三个白色面共用一个顶点,有8×8=64种方式;如果其中有两个白色面相对且通过另一个白色面连接,有12×12=144种方式;(5)由对称性,两个立方体均为全白、5白1黑、4白2黑的情况,与前三种情况一样.综上可得,两个立方体外观上完全相同的方式有2×(1+36+153)+208=588种,所求概率为588212=1471024,故(D)正确.21.有多少个正整数满足n+100070=⌊√n⌋?(注意⌊x⌋表示不超过x的最大整数.)(A)2(B)4(C)6(D)30(E)32解设⌊√n⌋=k,{√n}=r,则k 1,0 r<1.于是n=√n2=(⌊√n⌋+{√n})2=k2+2kr+r2,且n=70k−1000,从而原方程可化为k2−70k+1000+2kr+r2=0,即(k−20)(k−50)+2kr+r2=0.当r=0时,解得k=20,n=400,k=50,n=2500;当r>0时,解得k=21,n=470,k=49,n=2430,k=48,n=2360,k=47,n=2290.故共有6组解,(C)正确.22.对于实数t ,(2t −3t )t4t的最大值是多少?(A)116(B)115(C)112(D)110(E)19解令f (t )=(2t −3t )t 4t ,则f (t )=t 2t −3t 24t=t 2t −3·t 24t =y −3y 2,其中y =t2t .此时f (t )=−3y 2+y =−3(y −16)2+112,当y =16时,f (t )max =112.实际上,y =16即2t =6t ,从函数图像上可以发现,此方程有一个根t ∈(0,1).故(C)正确.23.有多少个整数n 2,使得只要复数z 1,z 2,···,z n满足|z 1|=|z 2|=···=|z n |=1,且z 1+z 2+···+z n =0,则z 1,z 2,···,z n 一定等距地分布在复平面的单位圆上?(A)1(B)2(C)3(D)4(E)5解(1)当n =2时,z 1=−z 2,此时z 1,z 2关于原点对称,平分单位圆;(2)当n =3时,z 1+z 2+z 3=0,由于|z 1+z 2|2+|z 1−z 2|2=2|z 1|2+2|z 2|2=4,从而|z 1−z 2|2=4−|z 1+z 2|2=4−|−z 3|2=3,即|z 1−z 2|=√3;同理|z 2−z 3|=|z 3−z 1|=√3,此时z 1,z 2,z 3构成一个正三角形,三等分单位圆;(3)当n =4时,z 1=35+45i ,z 2=−35+45i ,z 3=−35−45i ,z 4=35−45i 为符合条件的复数,构成一个不等距长方形;同理,当n =4k 时,容易构造出多个类似的不等距长方形;当n =4k +2时,再加两个复数±1仍然符合条件,且不等距;(4)当n =5时,z 1=1,z 2=−12+√32i ,z 3=−12−√32i ,z 4=i ,z 5=−i 为符合条件的复数,构成一个不等距五边形;同理,当n =4k +1时,只要再添加若干个不等距长方形即可符合条件;当n =4k +3时,再加两个复数±1仍然符合条件,且不等距.综上可得,满足条件且等距分布的只有n =2,3两种情况,故(B)正确.24.设D (n )表示正整数n 写成乘积n =f 1·f 2···f k的方式数目,其中k 1,且f i 是严格大于1的整数,因子的排列顺序也考虑在内(也就是说,两种仅因子顺序不同的表示被算作是不一样的).比如,6可以表示成6,2·3,3·2,所以D (6)=3.则D (96)是多少?(A)112(B)128(C)144(D)172(E)184解96=3×25,分情况讨论:(1)1个因子:就是表示成96,1种方式;(2)2个因子:可以表示成3×32,6×16,12×8,24×4,48×2,每一种表示可以交换顺序,共10种方式;(3)3个因子:可以表示成3×2×16,3×4×8,6×2×8,6×4×4,12×2×4,24×2×2,因子的顺序可以重新排列,共有P 33×4+C 13×2=30种方式;(4)4个因子:可以表示成3×2×2×8,3×2×4×4,6×2×2×4,12×2×2×2,重新排列后共有P 44÷2×3+C 14=40种方式;(5)5个因子:可以表示成3×2×2×2×4,6×2×2×2×2,重新排列后共有P 55÷P 33+C 15=25种方式;(6)6个因子:可以表示成3×2×2×2×2×2,重新排列后共有C 16=6种方式.由加法原理,可得112种方式,故(A)正确.25.对于每个实数0a <1,令x,y 为分别从区间[0,a ]和[0,1]中独立、随机地选取出来的数,且令P (a )为sin 2(πx )+sin 2(πy )>1成立的概率.则P (a )的最大值是多少?(A)712(B)2−√2(C)1+√24(D)√5−12(E)58解1<sin 2(πx )+sin 2(πy )=1−cos 2πx 2+1−cos 2πy2=1−12(cos 2πx +cos 2πy )=1−cos (x +y )π·cos (x −y )π,可得cos (x +y )π·cos (x −y )π<0,由于0 x a <1,0 y 1,从而x +y ∈[0,1+a ],x −y ∈[−1,a ].于是,不等式的解集为x +y ∈(0,12)∪(32,2)x −y ∈(−1,−12)∪(12,1)或x +y ∈(12,32),x −y ∈(−12,12).如图示,在直角坐标系中,符合条件的区域为五边形ABCDE .因此,所求概率P (a )=S ABCDE S OHMN =12−(1−a )21·a =2−(a +12a ) 2−√2,此时a =√22.故(B)正确.(注:为了节省版面,这里未提供英文版试题原文,对此有需要的读者,可联系编辑部向作者索取.)。
2023 amc12a 题

2023 amc12a 题2023 AMC 12A 题2023年的AMC 12A竞赛将是一场激烈的数学比赛,考察学生在各个领域的数学知识和解题能力。
以下是该竞赛中的一些题目及解答。
1. 第一题:一只兔子从森林的A点出发,每分钟能跑5米,而一只狐狸从森林的B点出发,每分钟能跑7米。
如果两只动物同时开始跑,那么它们跑到一起需要多长时间?解答:设它们跑到一起的时间为t分钟。
那么兔子跑的距离为5t米,狐狸跑的距离为7t米。
由于它们跑到一起,所以跑的距离相等,即5t=7t。
解这个方程得到t=0,这个结果是不合理的。
所以两只动物永远无法跑到一起。
2. 第二题:有一条长为12的直线,点A和点B分别在这条直线的两端。
点C 和点D在这条直线上,且距离点A的距离分别为2和6,距离点B的距离分别为3和9。
如果点A、B、C和D两两连线的长度之和为24,那么点C和点D之间的距离是多少?解答:设点C和点D之间的距离为x。
由题目可知,点A到点C的距离为2,点A到点D的距离为6,点B到点C的距离为3,点B到点D的距离为9。
根据直线的性质,我们知道点C到点D的距离等于点A到点B的距离减去点A到点C 的距离再减去点B到点D的距离。
即x=12-2-9=1。
所以点C和点D之间的距离为1。
3. 第三题:将正整数1到100分成若干个集合,使得每个集合中的两个数的差的绝对值不超过20。
求所分的集合的最少个数。
解答:我们可以考虑将这100个数分成5个集合,每个集合的范围为20个数。
即第一个集合为1到20,第二个集合为21到40,以此类推。
这样,任意两个数的差的绝对值不超过20。
所以所分的集合的最少个数为5个。
4. 第四题:在平面直角坐标系中,点A的坐标为(1, 3),点B的坐标为(4, 7)。
点C在x轴上,且点C到点A和点C到点B的距离相等。
求点C的坐标。
解答:设点C的坐标为(x, 0)。
由题目可知,点C到点A的距离等于点C到点B的距离。
根据距离的公式,我们可以得到√((x-1)^2+3^2)=√((x-4)^2+7^2)。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2002 AMC 12A ProblemsProblem 1Compute the sum of all the roots ofProblem 2Cindy was asked by her teacher to subtract 3 from a certain number and then divide the result by 9. Instead, she subtracted 9 and then divided the result by 3, giving an answer of 43. What would her answer have been had she worked the problem correctly?Problem 3According to the standard convention for exponentiation,If the order in which the exponentiations are performed is changed, how many other values are possible?Problem 4Find the degree measure of an angle whose complement is 25% of its supplement.Problem 5Each of the small circles in the figure has radius one. The innermost circle is tangent to the six circles that surround it, and each of those circles is tangent to the large circle and to its small-circle neighbors. Find the area of the shaded region.Problem 6For how many positive integers does there exist at least one positive integer n suchthat ?infinitely manyProblem 7A arc of circle A is equal in length to a arc of circle B. What is the ratio of circle A's area and circle B's area?Problem 8Betsy designed a flag using blue triangles, small white squares, and a red center square, as shown.Let be the total area of the blue triangles, the total area of the white squares,and the area of the red square. Which of the following is correct?Jamal wants to save 30 files onto disks, each with 1.44 MB space. 3 of the files take up 0.8 MB, 12 of the files take up 0.7 MB, and the rest take up 0.4 MB. It is not possible to split a file onto 2 different disks. What is the smallest number of disks needed to store all 30 files?Problem 10Sarah places four ounces of coffee into an eight-ounce cup and four ounces of cream into a second cup of the same size. She then pours half the coffee from the first cup to the second and, after stirring thoroughly, pours half the liquid in the second cup back to the first. What fraction of the liquid in the first cup is now cream?Problem 11Mr. Earl E. Bird gets up every day at 8:00 AM to go to work. If he drives at an average speed of 40 miles per hour, he will be late by 3 minutes. If he drives at an average speed of 60 miles per hour, he will be early by 3 minutes. How many miles per hour does Mr. Bird need to drive to get to work exactly on time?Problem 12Both roots of the quadratic equation are prime numbers. The number ofpossible values of isProblem 13Two different positive numbers and each differ from their reciprocals by . Whatis ?For all positive integers , let .Let . Which of the following relations is true?Problem 15The mean, median, unique mode, and range of a collection of eight integers are all equal to 8. The largest integer that can be an element of this collection isProblem 16Tina randomly selects two distinct numbers from the set {1, 2, 3, 4, 5}, and Sergio randomly selects a number from the set {1, 2, ..., 10}. What is the probability that Sergio's number is larger than the sum of the two numbers chosen by Tina?Problem 17Several sets of prime numbers, such as use each of the nine nonzero digits exactly once. What is the smallest possible sum such a set of primes could have?Problem 18Let and be circles definedby and respectively. What is the length ofthe shortest line segment that is tangent to at and to at ?The graph of the function is shown below. How many solutions does theequation have?Problem 20Suppose that and are digits, not both nine and not both zero, and the repeatingdecimal is expressed as a fraction in lowest terms. How many different denominators are possible?Problem 21Consider the sequence of numbers: For , the -th term of thesequence is the units digit of the sum of the two previous terms. Let denote the sum of thefirst terms of this sequence. The smallest value of for which is:Problem 22Triangle is a right triangle with as its rightangle, , and . Let be randomly chosen inside ,and extend to meet at . What is the probability that ?Problem 23In triangle , side and the perpendicular bisector of meet in point ,and bisects . If and , what is the area oftriangle ?Problem 1In the year, the United States will host the International Mathematical Olympiad.Let and be distinct positive integers such that the product .What is the largest possible value of the sum ?Problem 2Problem 3Each day, Jenny ate of the jellybeans that were in her jar at the beginning of that day.At the end of the second day, remained. How many jellybeans were in the jar originally?Problem 4The Fibonacci sequence starts with two 1s, and each term afterwards is the sum of its two predecessors. Which one of the ten digits is the last to appear in the units position of a number in the Fibonacci sequence?Problem 5If where thenProblem 6Two different prime numbers between and are chosen. When their sum is subtracted from their product, which of the following numbers could be obtained?Problem 7How many positive integers have the property that is a positive integer?Problem 8Figures, , , and consist of , , , and non-overlapping squares. If thepattern continued, how many non-overlapping squares would there be in figure?Problem 9Mrs. Walter gave an exam in a mathematics class of five students. She entered the scores in random order into a spreadsheet, which recalculated the class average after each score was entered. Mrs. Walter noticed that after each score was entered, the average was always an integer. The scores (listed in ascending order) were 71,76,80,82, and 91. What was the last score Mrs. Walters entered?Problem 10The point is reflected in the -plane, then its image is rotatedby about the -axis to produce , and finally, is translated by 5 units in thepositive-direction to produce . What are the coordinates of ?Problem 11Two non-zero real numbers, and satisfy. Which of the following is apossible value of?Problem 12Let A, M, and C be nonnegative integers such that . What is the maximumvalue of + + + ?Problem 13One morning each member of Angela’s family drank an 8-ounce mixture of coffee with milk. The amounts of coffee and milk varied from cup to cup, but were never zero. Angela drank a quarter of the total amount of milk and a sixth of the total amount of coffee. How many people are in the family?Problem 14When the mean,median, and modeof the listare arranged in increasing order, they form a non-constant arithmetic progression. What is the sum of all possible real values of?Problem 15Let be a function for which . Find the sum of all values of forwhich.Problem 16A checkerboard of rows and columns has a number written in each square, beginning inthe upper left corner, so that the first row is numbered , the secondrow , and so on down the board. If the board is renumbered so that the left column,top to bottom, is , the second column and so on across the board, some squares have the same numbers in both numbering systems. Find the sum of the numbers in these squares (under either system).Problem 17A centered at has radius and contains the point . The segment is tangent tothe circle at and . If point lieson and bisects , thenProblem 18In year , the day of the year is a Tuesday. In year , the day isalso a Tuesday. On what day of the week did th day of year occur?Problem 19triangle , , , . Let denote the midpointof and let denote the intersection of with the bisector of angle .Which of the following is closest to the area of the triangle ?Problem 20If and are positive numbers satisfyingThen what is the value of latex ?Problem 21Through a point on the hypotenuse of right triangle, lines are drawn parallel to the legs of the triangle so that the triangle is divided into asquare and two smaller right triangles. The area of one of the two small right triangles times the area of the square. The ratio of the area of the other small right triangle to the area of the square isProblem 22The graph below shows a portion of the curve defined by the quarticpolynomial. Which of the following is the smallest?Problem 23Professor Gamble buys a lottery ticket, which requires that he pick six different integersfrom through , inclusive. He chooses his numbers so that the sum of the base-ten logarithms of his six numbers is an integer. It so happens that the integers on the winning ticket have the same property— the sum of the base-ten logarithms is an integer. What is the probability that Professor Gamble holds the winning ticket?Problem 24If circular arcs and centers at and , respectively, then there exists acircletangent to both and , and to . If the length of is , then the circumference of the circle isProblem 25Eight congruent Equilateral triangle each of a different color, are used to construct a regular octahedron. How many distinguishable ways are there to construct the octahedron? (Two colored octahedrons are distinguishable if neither can be rotated to look just like the other.)。
