AMC10美国数学竞赛A卷附中文翻译和答案之欧阳学创编
2010年北美数学建模竞赛中英文A、B题目

2010年北美数学建模比赛中英文题目(MCM)2010 MCM题目A题:棒球棒上的最佳击球点Explain the “sweet spot” on a baseball bat.Every hitter knows that there is a spot on the fat part of a baseball bat where maximum power is transferred to the ball when hit. Why isn’t this spot at the end of the bat? A simple explanation based on torque might seem to identify the end of the bat as the sweet spot, but this is known to be empirically incorrect. Develop a model that helps explain this empirical finding.Some players believe that “corking” a bat (hollowing out a cylinder in the head of the bat and filling it with cork or rubber, then replacing a wood cap) enhances the “sweet spot” effect. Augment your model to confirm or deny this effect. Does this explain why Major League Baseball prohibit s “corking”?Does the material out of which the bat is constructed matter? That is, does this model predict different behavior for wood (usually ash) or metal (usually aluminum) bats? Is this why Major League Baseball prohibits metal bats?中文翻译:解释棒球棒上的“最佳击球点”。
美国数学测评AMC10A-2019(中英双语)

1.What is the value of下方表达式的值是多少?(A) 0 (B) 1 (C) 2 (D) 3 (E) 42.What is the hundreds digit of (20! − 15!) ? (20! − 15!)的百位上的数字是多少? (A) 0 (B) 1 (C) 2 (D) 4(E) 53.Ana and Bonita were born on the same date in different years, n years apart. Last year Ana was 5 times as old as Bonita. This year Ana’s age is the square of Bonita’s age. What is n ?Ana 和Bonita 出生在不同年份的同一天, 相隔n 年。
去年Ana 的年龄是Bonita 年龄的5倍。
今年Ana 的年龄是Bonita 年龄的平方。
问n 的值是多少?(A) 3 (B) 5 (C) 9 (D) 12 (E) 154.A box contains 28 red balls, 20 green balls, 19 yellow balls, 13 blue balls, 11 white balls, and 9 black balls. What is the minimum number of balls that must be drawn from the box without replacement to guarantee that at least 15 balls of a single color will be drawn?一个盒子中有28个红球,20个绿球,19个黄球,13个蓝球,11个白球和9个黑球。
为了保证至少取出15个单一颜色的球, 在不允许放回重取的情况下,须从盒子里至少取出多少个球?(A) 75(B) 76(C) 79(D) 84(E) 915.What is the greatest number of consecutive integers whose sum is 45 ? 最多可以有多少个连续整数的和是45?(A) 9 (B) 25 (C) 45 (D) 90 (E) 1206. For how many of the following types of quadrilaterals does there exist a point in the plane of thequadrilateral that is equidistant from all four vertices of the quadrilateral?2019 AMC 10A Problems • a square• a rectangle that is not a square• a rhombus that is not a square• a parallelogram that is not a rectangle or a rhombus•an isosceles trapezoid that is not a parallelogram 在以下类型的四边形中,有多少种在平面上存在一个与其所有四个顶点距离都相等的点?• 正方形• 不是正方形的长方形• 不是正方形的菱形• 不是长方形或菱形的平行四边形• 不是平行四边形的等腰梯形(A) 1(B) 2 (C) 3 (D) 4 (E) 57. Two lines with slopes and intersect at . What is the area of the triangle enclosed by these two lines and the line两条斜率分别为1/2和2的直线相交于(2, 2)。
2019年美国数学竞赛(AMC10A)的试题与解答

(
)
0(19 ) 译文: 2
+ ((20)1)9 的值是多少?
解 原式 = 20 + 19 = 2, 故 (C) 正确.
2. What is the hundreds digit of (20! − 15!)?
(A) 0 (B) 1 (C) 2 (D) 4 (E) 5
译文: (20! − 15!) 的百位数是多[ 少]?
years, n years apart. year Ana was 5 times as old as Bonita.
This year Ana’s age is the square of Bonita’s age. What is n?
(A) 3 (B) 5 (C) 9 (D) 12 (E) 15
equidistant from all four vertices of the quadrilateral?
— a square
— a rectangle that is not a square
— a rhombus that is not a square
— a parallelogram that is not a rectangle or a rhombus
13 个蓝球, 11 个白球和 9 个黑球. 在不放回的情况下, 必须
至少从盒子中取出多少个球才能确保会取出 15 个同种颜色
的球?
解 依题意, 红球、绿球、黄球得取出 14 个, 蓝球、白球、
黑球全部取出, 再加任意 1 个就符合条件, 即至少需要取出
14 × 3 + 13 + 11 + 9 + 1 = 76 个球, 故 (B) 正确.
2011年-AMC10数学竞赛A卷-附中文翻译和答案

2011年A M C1 0美国数学竞赛A 卷1. A cell phone plan costs $20 each month, plus 5¢per text message sent, plus 10¢for each minute used over 30 hours. In January Michelle sent 100 text messages and talked for 30.5 hours. How much did she have to pay?(A) $24.00 (B) $24.50 (C) $25.50 (D) $28.00 (E) $30.002. A small bottle of shampoo can hold 35 milliliters of shampoo, Whereas a large bottle can hold 500 milliliters of shampoo. Jasmine wants to buy the minimum number of small bottles necessary to completely fill a large bottle. How many bottles must she buy?(A) 11 (B) 12 (C) 13 (D) 14 (E) 153. Suppose [a b] denotes the average of a and b, and {a b c} denotes the average of a, b, and c. What is {{1 1 0} [0 1] 0}?(A) 29(B)518(C)13(D) 718(E) 234. Let X and Y be the following sums of arithmetic sequences: X= 10 + 12 + 14 + … + 100.Y= 12 + 14 + 16 + … + 102.What is the value of Y X?(A) 92 (B) 98 (C) 100 (D) 102(E) 1125. At an elementary school , the students in third grade, fourth grade, and fifth grade run an average of 12, 15 , and 10 minutes per day, respectively. There are twice as many third graders as fourth graders, and twice as many fourth graders as fifth graders. What is the average number of minutes run per day by these students?(A) 12 (B) 373 (C) 887 (D) 13 (E) 146. Set A has 20 elements, and set B has 15 elements. What is the smallest possible number of elements in A ∪B, the union of A and B?(A) 5 (B) 15 (C) 20 (D) 35 (E) 3007. Which of the following equations does NOT have a solution?(A) 2(7)0x += (B) -350x += (C) 20=(D) 80= (E) -340x -=8. Last summer 30% of the birds living on Town Lake were geese, 25% were swans, 10% were herons , and 35% were ducks. What percent of the birds that were not swans were geese?(A) 20 (B) 30 (C) 40 (D) 50 (E) 609. A rectangular region is bounded by the graphs of the equations y=a, y=-b, x=-c, and x=d, where a, b, c, and d are all positive numbers. Which of the following represents the area of this region?(A) ac + ad + bc + bd(B) ac – ad + bc – bd (C) ac + ad – bc – bd(D) –ac –ad + bc + bd (E) ac – ad – bc + bd10. A majority of the 20 students in Ms. Deameanor’s class bought pencils at the school bookstore. Each of these students bought the same number of pencils, and this number was greater than 1. The cost of a pencil in cents was greater than the number of pencils each student bought, and the total cost of all the pencils was $17.71. What was the cost of a pencil in cents?(A) 7 (B) 11 (C) 17 (D) 23 (E) 7711. Square EFGH has one vertex on each side of square ABCD. Point E is on AB with AE=7·EB. What is the ratio of the area of EFGH to the area of ABCD?(A) 4964 (B) 2532 (C) 78 (D) 8 (E)12. The players on a basketball team made some three-point shots, some two-point shots, some one-point free t hrows. They scored as many points with two-point shots as with three-point shots. Their number of successful free throws was one more than their number of successful t wo-point shots. The team’s total score was 61 points. Howmany free throws did they m ake?(A) 13 (B) 14 (C) 15 (D) 16 (E) 1713. How many even integers are there between 200 and 700 whose digits are all different and come from the set {1, 2, 5, 7, 8, 9}?(A) 12 (B)20 (C)72 (D) 120 (E) 20014. A pair of standard 6-sided fai r dice is rolled once. The sum of the numbers rolled determines the diameter of a circle . What is the probability that the numerical value of the area of the circle is less than th e numerical value of the circle’s circumference?(A) 136(B)112(C)16(D) 14(E) 5181 5. Roy bought a new battery-gasoline hybrid car. On a trip the car ran exclusively on I ts battery for the first 40 miles, then ran exclusively on gasoline for the rest of the trip, using gasoline at a rate of 0.02 gallons per mile. On the whole trip he averaged 55 m iles per gallon. How long was the trip in miles?(A) 140 (B) 240 (C) 440 (D) 640 (E) 84016. Wh ich of the following in equal to(A) (B) (C) 2 (D) (E) 617. In the eight-term sequence A, B, C, D, E, F, G, H, the value of C is 5 and the sum o f any three consecutive terms is30. What is A + H?(A) 17 (B) 18 (C) 25 (D) 26 (E) 431 8. Circles A, B, and C each have radius 1. Circles A and B share one point of ta ngency. Circle C has a point of tangency with the midpoint of AB. What is the area inside Circle C but outside Circle A and Circle B? (A) 32π- (B) 2π (C) 2 (D) 34π (E) 12π+1 9. In 1991 the population of a town was a perfect square. Ten years later, after an in crease of 150 people, the population was 9 more than a perfect square. Now, in 2011, with an increase of another 150 people, the population is once again a perfect square. Which of the following is closest to the percent growth of the town’s population during this twenty-year period?(A) 42 (B) 47 (C) 52 (D) 57 (E) 622 0. Two points on the circumference of a circle of radius r are selected independently an d at random. From each point a chord of length r is drawn in a clockwise direction. Wh at is the probability that the two chords intersect?(A) 16(B) 15(C) 14(D) 13(E) 122 1. Two counterfeit coins of equal weight are mixed with 8 identical genuine coins. T he weight of each of the counterfeit coins is different from the weight of each of the genuine coins. A pair of coins is selected at random without replacement from the 10 coins. A second pair is selected at random without replacement from the remaining 8 coins. The combined weight of the first pair is equal to the combined weight of the second pair. What is the probability that all 4 selected coins are genuine?(A) 711(B) 913(C) 1115(D) 1519(E) 15162 2. Each vertex of convex pentagon ABCDE is to be assigned a color. There are 6 co lors to choose from, and the ends of each diagonal must have different colors. How many different colorings are possible?(A) 2500 (B) 2880 (C) 3120 (D) 3250 (E) 37502 3. Seven students count from 1 to 1000 as follows:·Alice says all the numbers, except she skips the middle number in each consecutive group of three numbers. That is Alice says 1, 3, 4, 6, 7, 9, …, 997, 999, 1000.·Barbara says all of the numbers that Alice doesn’t say, except she also skips the middle number in each consecutive grope of three numbers.·Candice says all of the numbers that neither Alice nor Barbara says, except she also skips the middlenumber in each consecutive group of three numbers.· Debbie, Eliza, and Fatima say all of the numbers that none of the students with the first names beginning before theirs in the alphabet say, except each also skips the middle number in each of her consecutive groups of three numbers.· Finally, George says the only number that no one else says.Wh at number does George say?(A) 37 (B) 242 (C) 365 (D) 728 (E) 9982 4. Two distinct regular tetrahedra have all their vertices among the vertices of the sa me unit cube. What is the volume of the region formed by the intersection of the tetrahedra?(A) 112 (B) 12 (C) (D)16 (E) 62 5. Let R be a square region and 4n an integer. A point X in the interior of R is ca lled n-ray partitional if there are n rays emanating from X that divide R into N triangles of equal area. How many points are 100-ray partitional but not 60-ray partitional?(A) 1500 (B) 1560 (C) 2320 (D) 2480 (E) 25002011AMC10美国数学竞赛A卷1. 某通讯公司手机每个月基本费为20美元, 每传送一则简讯收5美分(一美元=100 美分)。
2000-2012美国AMC10中文版试题及答案

2000到2012年AMC10美国数学竞赛0 0P 0 A 0 B 0 C 0D 0 全美中学数学分级能力测验(AMC 10)2000年 第01届 美国AMC10 (2000年2月 日 时间75分钟)1. 国际数学奥林匹亚将于2001年在美国举办,假设I 、M 、O 分别表示不同的正整数,且满足I ⨯M ⨯O =2001,则试问I +M +O 之最大值为 。
(A) 23 (B) 55 (C) 99 (D) 111 (E) 6712. 2000(20002000)为 。
(A) 20002001 (B) 40002000 (C) 20004000 (D) 40000002000 (E) 200040000003. Jenny 每天早上都会吃掉她所剩下的聪明豆的20%,今知在第二天结束时,有32颗剩下,试问一开始聪明豆有 颗。
(A) 40 (B) 50 (C) 55 (D) 60 (E) 754. Candra 每月要付给网络公司固定的月租费及上网的拨接费,已知她12月的账单为12.48元,而她1月的账单为17.54元,若她1月的上网时间是12月的两倍,试问月租费是 元。
(A) 2.53 (B) 5.06 (C) 6.24 (D) 7.42 (E) 8.775. 如图M ,N 分别为PA 与PB 之中点,试问当P 在一条平行AB 的直 在线移动时,下列各数值有 项会变动。
(a) MN 长 (b) △P AB 之周长 (c) △P AB 之面积 (d) ABNM 之面积(A) 0项 (B) 1项 (C) 2项 (D) 3项 (E) 4项 6. 费氏数列是以两个1开始,接下来各项均为前两项之和,试问在费氏数列各项的个位数字中, 最后出现的阿拉伯数字为 。
(A) 0 (B) 4 (C) 6 (D) 7 (E) 97. 如图,矩形ABCD 中,AD =1,P 在AB 上,且DP 与DB 三等分∠ADC ,试问△BDP 之周长为 。
2011AMC10美国数学竞赛A卷附中文翻译和答案

2011A M C10美国数学竞赛A卷附中文翻译和答案2011AMC10美国数学竞赛A卷1. A cell phone plan costs $20 each month, plus 5¢ per text message sent, plus 10¢ for each minute used over 30 hours. In January Michelle sent 100 text messages and talked for 30.5 hours. How much did she have to pay?(A) $24.00 (B) $24.50 (C) $25.50 (D) $28.00 (E) $30.002. A small bottle of shampoo can hold 35 milliliters of shampoo, Whereas a large bottle can hold 500 milliliters of shampoo. Jasmine wants to buy the minimum number of small bottles necessary to completely fill a large bottle. How many bottles must she buy?(A) 11 (B) 12 (C) 13 (D) 14 (E) 153. Suppose [a b] denotes the average of a and b, and {a b c} denotes the average of a, b, and c. What is {{1 1 0} [0 1] 0}?(A) 29(B)518(C)13(D) 718(E) 234. Let X and Y be the following sums of arithmetic sequences:X= 10 + 12 + 14 + …+ 100.Y= 12 + 14 + 16 + …+ 102.What is the value of Y X?(A) 92 (B) 98 (C) 100 (D) 102 (E) 1125. At an elementary school, the students in third grade, fourth grade, and fifth grade run an average of 12, 15, and 10 minutes per day, respectively. There are twice asmany third graders as fourth graders, and twice as many fourth graders as fifth graders. What is the average number of minutes run per day by these students?(A) 12(B) 373 (C) 887 (D) 13 (E) 146. Set A has 20 elements, and set B has 15 elements. What is the smallest possible number of elements in A ∪B, the union of A and B?(A) 5(B) 15 (C) 20 (D) 35 (E) 3007. Which of the following equations does NOT have a solution?(A)2(7)0x +=(B) -350x += 20=80=(E) -340x -=8. Last summer 30% of the birds living on Town Lake were geese, 25% were swans, 10% were herons, and 35% were ducks. What percent of the birds that were not swans were geese?(A) 20(B) 30 (C) 40 (D) 50 (E) 609. A rectangular region is bounded by the graphs of the equations y=a, y=-b, x=-c, and x=d, where a, b, c, and d are all positive numbers. Which of the following represents the area of this region?(A) ac + ad + bc + bd(B) ac – ad + bc – bd (C) ac + ad – bc – bd (D) –ac –ad + bc + bd(E) ac – ad – bc + bd10. A majority of the 20 students in Ms. Deameanor’s class bought pencils at theschool bookstore. Each of these students bought the same number of pencils, and this number was greater than 1. The cost of a pencil in cents was greater than the number of pencils each student bought, and the total cost of all the pencils was $17.71. What was the cost of a pencil in cents?(A) 7(B) 11 (C) 17 (D) 23 (E) 7711. Square EFGH has one vertex on each side of square ABCD. Point E is on AB with AE=7·EB. What is the ratio of the area of EFGH to the area of ABCD?(A)4964 (B) 2532 (C) 78 (D) 8 (E) 412. The players on a basketball team made some three-point shots, some two-pointshots, some one-point free throws. They scored as many points with two-point shots as with three-point shots. Their number of successful free throws was one more than their number of successful two-point shots. The team’s total score was 61 points. Howmany free throws did they make?(A) 13 (B) 14 (C) 15 (D) 16 (E) 1713. How many even integers are there between 200 and 700 whose digits are all different and come from the set {1, 2, 5, 7, 8, 9}?(A) 12 (B)20 (C)72 (D) 120 (E) 20014. A pair of standard 6-sided fair dice is rolled once. The sum of the numbers rolled determines the diameter of a circle. What is the probability that the numerical value of the area of the circle is less than the numerical value of the circle’s circumference?(A) 136(B)112(C)16(D) 14(E) 51815. Roy bought a new battery-gasoline hybrid car. On a trip the car ran exclusively on its battery for the first 40 miles, then ran exclusively on gasoline for the rest of the trip, using gasoline at a rate of 0.02 gallons per mile. On the whole trip he averaged55 miles per gallon. How long was the trip in miles?(A) 140 (B) 240 (C) 440 (D) 640 (E) 84016. Which of the following in equal to(A)(B) (D) (E) 617. In the eight-term sequence A, B, C, D, E, F, G, H, the value of C is 5 and the sum of any three consecutive terms is 30. What is A + H?(A) 17(B) 18 (C) 25 (D) 26 (E) 4318. Circles A, B, and C each have radius 1. Circles A and B share one point oftangency. Circle C has a point of tangency with the midpoint of AB. What is the area inside Circle C but outside Circle A and Circle B? (A) 32π- (B) 2π(C) 2 (D) 34π (E) 12π+19. In 1991 the population of a town was a perfect square. Ten years later, after anincrease of 150 people, the population was 9 more than a perfect square. Now, in 2011, with an increase of another 150 people, the population is once again a perfect square. Which of the following is closest to the pe rcent growth of the town’s populationduring this twenty-year period?(A) 42(B) 47 (C) 52 (D) 57 (E) 6220. Two points on the circumference of a circle of radius r are selected independently and at random. From each point a chord of length r is drawn in a clockwise direction. What is the probability that the two chords intersect? (A) 16 (B) 15 (C) 14 (D) 13 (E) 1221. Two counterfeit coins of equal weight are mixed with 8 identical genuine coins. The weight of each of the counterfeit coins is different from the weight of each of the genuine coins. A pair of coins is selected at random without replacement from the 10coins. A second pair is selected at random without replacement from the remaining 8 coins. The combined weight of the first pair is equal to the combined weight of the second pair. What is the probability that all 4 selected coins are genuine?(A) 711(B) 913(C) 1115(D) 1519(E) 151622. Each vertex of convex pentagon ABCDE is to be assigned a color. There are 6 colors to choose from, and the ends of each diagonal must have different colors. How many different colorings are possible?(A) 2500 (B) 2880 (C) 3120 (D) 3250 (E) 375023. Seven students count from 1 to 1000 as follows:·Alice says all the numbers, except she skips the middle number in each consecutive group of three numbers. That is Alice says 1, 3, 4, 6, 7, 9, …, 997, 999, 1000.·Barbara says all of the numbers that Alice doesn’t say, except she also skips the middle number in each consecutive grope of three numbers.·Candice says all of the numbers that neither Alice nor Barbara says, except she also skips the middle number in each consecutive group of three numbers. ·Debbie, Eliza, and Fatima say all of the numbers that none of the students with the first names beginning before theirs in the alphabet say, except each also skips the middle number in each of her consecutive groups of three numbers.·Finally, George says the only number that no one else says.What number does George say?(A) 37(B) 242 (C) 365 (D) 728 (E) 99824. Two distinct regular tetrahedra have all their vertices among the vertices of the same unit cube. What is the volume of the region formed by the intersection of the tetrahedra?(A)112 (B) 12 (C) 12 (D) 16 (E) 625. Let R be a square region and 4n an integer. A point X in the interior of R is called n-ray partitional if there are n rays emanating from X that divide R into Ntriangles of equal area. How many points are 100-ray partitional but not 60-raypartitional?(A) 1500(B) 1560 (C) 2320 (D) 2480 (E) 25002011AMC10美国数学竞赛A 卷1. 某通讯公司手机每个月基本费为20美元, 每传送一则简讯收 5美分(一美元=100 美分)。
USA 2010 AMC 10 A 中文翻译及答案

USA 2010 A1. 小华的书架顶上的5本书的厚度分别是:, , , , 公分,请问这些书的平均厚度是多少公分?(A)1 (B)2 (C)3 (D)4 (E)52. 四个相同的小正方形和一个长方形形成一个大正方形,如下所示。
长方形中长是宽的多少倍?3. 小明有97颗弹珠和小钟有11颗弹子。
然后小明把他的一些弹珠给了小钟,所以小明有小钟2倍的弹珠。
小明给了小钟多少弹珠?(A)3 (B)13 (C)18 (D)25 (E)294.某本书转录成有声书需要报读412分钟,现在要将它储存在光盘片里。
已知每片光盘最多可以储存报读时间为56分钟,假设用最少片的光盘,使得每片光盘所储存报读的时间都相同。
试问每片光盘应该储存报读的时间是多少分钟?(A)50.2 (B)51.5 (C)52.4 (D)53.8 (E)55.25.若圆周长为之圆的面积为,那么K数值是多少?(A)6 (B)12 (C)24 (D)36 (E)1446. 对于正数X,Y,定义运算如下:。
试问是多少?7.小美每天一招固定路线跑步,她首先朝正北跑了1公里,然后她跑东北方向1公里,然后东南方向1公里。
她最后按照直线方向跑回原出发点。
她最后一段跑了多少公里?8.小明一天工作2小时,每小时工资0. 50美元乘以他工作时的年龄。
他在六个月的期间,一共工作了50天,共赚630美元。
试问他在六个月工作结束时年龄是多少岁?9.如果一个数由左至右念与由右至左念都一样,我们称它为回文数,例如83438,已知X与 X+32分别为三位数与四位数的回文数,试问X各位数字的总和是多少?10.小华的生日是5月27日,在闰年2008年的生日是星期二。
试问未来他的生日在星期六的最近一年是哪一年?11. 设不等式解的范围之长度为.试问是多少?12.小罗按照比例缩小尺寸制作他所居住城镇的模型。
城镇的水塔高40公尺,顶部是一个球形储水槽,拥有100000公升的水。
若小罗模型水塔的储水槽可存0.1升。
2011AMC10美国数学竞赛A卷 中文翻译及答案

2011AMC10美国数学竞赛A卷1. 某通讯公司手机每个月基本费为20美元, 每传送一则简讯收 5美分(一美元=100 美分)。
若通话超过30小时,超过的时间每分钟加收10美分。
已知小美一月份共传送了100条简讯及通话30.5小时,则她需要付多少美元?(A) $24.00 (B) $24.50 (C) $25.50 (D) $28.00 (E) $30.002.小瓶装有35毫升的洗发液,大瓶可装500毫升的洗发液。
小华至少要买多少瓶小瓶的洗发液才能装满一个大瓶的洗发液?(A) 11 (B) 12 (C) 13 (D) 14 (E) 153. 若以 [a b]表示 a , b两数的平均数, 以 {a b c} 表示a, b, c三数的平均数,则{{1 1 0} [0 1] 0}之值为何?(A) 29(B)518(C)13(D) 718(E) 234. 设 X 和 Y 为下列等差级数之和:X= 10 + 12 + 14 + …+ 100.Y= 12 + 14 + 16 + …+ 102.则Y X之值为何?(A) 92 (B) 98 (C) 100 (D) 102 (E) 1125. 在某小学三年级,四年级及五年级的学生,每天分别平均跑12, 15, 及10 分钟, 已知三年级的学生人数是四年级人数的两倍,四年级的学生人数是五年级学生人数的两倍。
试问所有这些学生每天平均跑几分钟?(A) 12 (B) 373 (C) 887 (D) 13 (E) 146. 已知集合A 中有20个元素, 集合B 中有 15 个元素. A ∪B 是集合A 和集合B 的联集,它是由集合A 与集合B 中所有元素所形成的集合,则集合A ∪B 中至少有多少个元素?(A) 5(B) 15 (C) 20 (D) 35 (E) 3007.下列哪个方程式没有解?(A) 2(7)0x += (B) -350x += 20=80= (E) -340x -=8.去年夏季保护区里有 30%是鹅 ,25%是鸳鸯, 10%是苍鹰, 35% 是鸭子. 试问不是鸳鸯的鸟类中鹅占多少百分比?(A) 20(B) 30 (C) 40 (D) 50 (E) 609. 某个矩形是由y=a, y=-b, x=-c, 与x=d,的圆形所围成的,其中a, b, c, , d 均为正数。
美国AMC10中文版试题及答案

2000到20XX年AMC10美国数学竞赛0 0P 0 A 0 B 0 C 0D 0 全美中学数学分级能力测验(AMC 10)2000年 第01届 美国AMC10 (2000年2月 日 时间75分钟)1. 国际数学奥林匹亚将于 在美国举办,假设I 、M 、O 分别表示不同的正整数,且满足I ⨯M ⨯O =2001,则试问I +M +O 之最大值为 。
(A) 23 (B) 55 (C) 99 (D) 111 (E) 6712. 2000(20002000)为 。
(A) 20002001 (B) 40002000 (C) 20004000 (D) 40000002000 (E) 200040000003. Jenny 每天早上都会吃掉她所剩下的聪明豆的20%,今知在第二天结束时,有32颗剩下,试问一开始聪明豆有 颗。
(A) 40 (B) 50 (C) 55 (D) 60 (E) 754. Candra 每月要付给网络公司固定的月租费及上网的拨接费,已知她12月的账单为12.48元,而她1月的账单为17.54元,若她1月的上网时间是12月的两倍,试问月租费是 元。
(A) 2.53 (B) 5.06 (C) 6.24 (D) 7.42 (E) 8.775. 如图M ,N 分别为PA 与PB 之中点,试问当P 在一条平行AB 的直 在线移动时,下列各数值有 项会变动。
(a) MN 长 (b) △P AB 之周长 (c) △P AB 之面积 (d) ABNM 之面积(A) 0项 (B) 1项 (C) 2项 (D) 3项 (E) 4项 6. 费氏数列是以两个1开始,接下来各项均为前两项之和,试问在费氏数列各项的个位数字中, 最后出现的阿拉伯数字为 。
(A) 0 (B) 4 (C) 6 (D) 7 (E) 97. 如图,矩形ABCD 中,AD =1,P 在AB 上,且DP 与DB 三等分∠ADC ,试问△BDP 之周长为 。
2011AMC10美国数学竞赛A卷附中文翻译和答案

2011AMC10美国数学竞赛A卷1. A cell phone plan costs $20 each month, plus 5¢ per text message sent, plus 10¢ for each minute used over 30 hours. In January Michelle sent 100 text messages and talked for 30.5 hours. How much did she have to pay?(A) $24.00 (B) $24.50 (C) $25.50 (D) $28.00 (E) $30.002. A small bottle of shampoo can hold 35 milliliters of shampoo, Whereas a large bottle can hold 500 milliliters of shampoo. Jasmine wants to buy the minimum number of small bottles necessary to completely fill a large bottle. How many bottles must she buy?(A) 11 (B) 12 (C) 13 (D) 14 (E) 153. Suppose [a b] denotes the average of a and b, and {a b c} denotes the average of a, b, and c. What is {{1 1 0} [0 1] 0}?(A) 29(B)518(C)13(D) 718(E) 234. Let X and Y be the following sums of arithmetic sequences: X= 10 + 12 + 14 + …+ 100.Y= 12 + 14 + 16 + …+ 102.What is the value of Y X?(A) 92 (B) 98 (C) 100 (D) 102 (E) 1125. At an elementary school, the students in third grade, fourth grade, and fifth grade run an average of 12, 15, and 10 minutes per day, respectively. There are twice as many third graders as fourth graders, and twice as many fourth graders as fifth graders. What is the average number of minutes run per day by these students?(A) 12 (B) 373 (C) 887 (D) 13 (E) 146. Set A has 20 elements, and set B has 15 elements. What is the smallest possible number of elements in A ∪B, the union of A and B?(A) 5(B) 15 (C) 20 (D) 35 (E) 3007. Which of the following equations does NOT have a solution?(A)2(7)0x +=(B) -350x += (C) 20= (D)80= (E) -340x -=8. Last summer 30% of the birds living on Town Lake were geese, 25% were swans, 10% were herons, and 35% were ducks. What percent of the birds that were not swans were geese?(A) 20(B) 30 (C) 40 (D) 50 (E) 609. A rectangular region is bounded by the graphs of the equations y=a, y=-b, x=-c, and x=d, where a, b, c, and d are all positive numbers. Which of the following represents the area of this region?(A) ac + ad + bc + bd (B) ac – ad + bc – bd (C) ac + ad – bc – bd(D) –ac –ad + bc + bd (E) ac – ad – bc + bd10. A majority of the 20 students in Ms. Deameanor’s class bought pencils at the school bookstore. Each of these students bought the same number of pencils, and this number was greater than 1. The cost of a pencil in cents was greater than the number of pencils each student bought, and the total cost of all the pencils was $17.71. What was the cost of a pencil in cents?(A) 7(B) 11 (C) 17 (D) 23 (E) 7711. Square EFGH has one vertex on each side of square ABCD. Point E is on AB with AE=7·EB. What is the ratio of the area of EFGH to the area of ABCD?(A)4964 (B) 2532 (C) 78 (D) (E)12. The players on a basketball team made some three-point shots, some two-point shots, some one-point free throws. They scored as many points with two-point shots as with three-point shots. Their number of successful free throws was one more than their number of successful two-point shots. The team’s total score was 61 points. How many free throws did they make?(A) 13(B) 14 (C) 15 (D) 16 (E) 1713. How many even integers are there between 200 and 700 whose digits are alldifferent and come from the set {1, 2, 5, 7, 8, 9}?(A) 12(B) 20 (C) 72 (D) 120 (E) 20014. A pair of standard 6-sided fair dice is rolled once. The sum of the numbers rolled determines the diameter of a circle. What is the probability that the numerical value of the area of the circle is less than the numerical value of the circle’s circumference? (A)136 (B) 112 (C) 16 (D) 14 (E) 51815. Roy bought a new battery-gasoline hybrid car. On a trip the car ran exclusively on its battery for the first 40 miles, then ran exclusively on gasoline for the rest of the trip, using gasoline at a rate of 0.02 gallons per mile. On the whole trip he averaged 55 miles per gallon. How long was the trip in miles?(A) 140(B) 240 (C) 440 (D) 640 (E) 84016. Which of the following in equal to(A)(B) (C) 2 (D) (E) 617. In the eight-term sequence A, B, C, D, E, F, G , H, the value of C is 5 and the sum of any three consecutive terms is 30. What is A + H?(A) 17(B) 18 (C) 25 (D) 26 (E) 4318. Circles A, B, and C each have radius 1. Circles A and B share one point oftangency. Circle C has a point of tangency with the midpoint of AB. What is the area inside Circle C but outside Circle A and Circle B? (A) 32π- (B) 2π (C) 2 (D) 34π (E) 12π+19. In 1991 the population of a town was a perfect square. Ten years later, after an increase of 150 people, the population was 9 more than a perfect square. Now, in 2011, with an increase of another 150 people, the population is once again a perfect square. Which of the following is closest to the percent growth of the town’s popu lation during this twenty-year period?(A) 42(B) 47 (C) 52 (D) 57 (E) 6220. Two points on the circumference of a circle of radius r are selected independently and at random. From each point a chord of length r is drawn in a clockwise direction. What is the probability that the two chords intersect? (A)16 (B) 15 (C) 14 (D) 13 (E) 1221. Two counterfeit coins of equal weight are mixed with 8 identical genuine coins. The weight of each of the counterfeit coins is different from the weight of each of the genuine coins. A pair of coins is selected at random without replacement from the 10 coins. A second pair is selected at random without replacement from the remaining 8 coins. The combined weight of the first pair is equal to the combined weight of the second pair. What is the probability that all 4 selected coins are genuine?(A) 711(B) 913(C) 1115(D) 1519(E) 151622. Each vertex of convex pentagon ABCDE is to be assigned a color. There are 6 colors to choose from, and the ends of each diagonal must have different colors. How many different colorings are possible?(A) 2500 (B) 2880 (C) 3120 (D) 3250 (E) 375023. Seven students count from 1 to 1000 as follows:·Alice says all the numbers, except she skips the middle number in each consecutive group of thre e numbers. That is Alice says 1, 3, 4, 6, 7, 9, …, 997, 999, 1000.·Barbara says all of the numbers that Alice doesn’t say, except she also skips the middle number in each consecutive grope of three numbers.·Candice says all of the numbers that neither Alice nor Barbara says, except she also skips the middle number in each consecutive group of three numbers. ·Debbie, Eliza, and Fatima say all of the numbers that none of the students with the first names beginning before theirs in the alphabet say, except each also skips the middle number in each of her consecutive groups of three numbers.·Finally, George says the only number that no one else says.What number does George say?(A) 37 (B) 242 (C) 365 (D) 728 (E) 99824. Two distinct regular tetrahedra have all their vertices among the vertices of thesame unit cube. What is the volume of the region formed by the intersection of the tetrahedra?(A)112 (B) (C) (D) 16 (E)25. Let R be a square region and 4n an integer. A point X in the interior of R is called n-ray partitional if there are n rays emanating from X that divide R into N triangles of equal area. How many points are 100-ray partitional but not 60-ray partitional?(A) 1500(B) 1560 (C) 2320 (D) 2480 (E) 25002011AMC10美国数学竞赛A 卷1. 某通讯公司手机每个月基本费为20美元, 每传送一则简讯收 5美分(一美元=100 美分)。
美国数学测评AMC10A-2020 (中英双语)
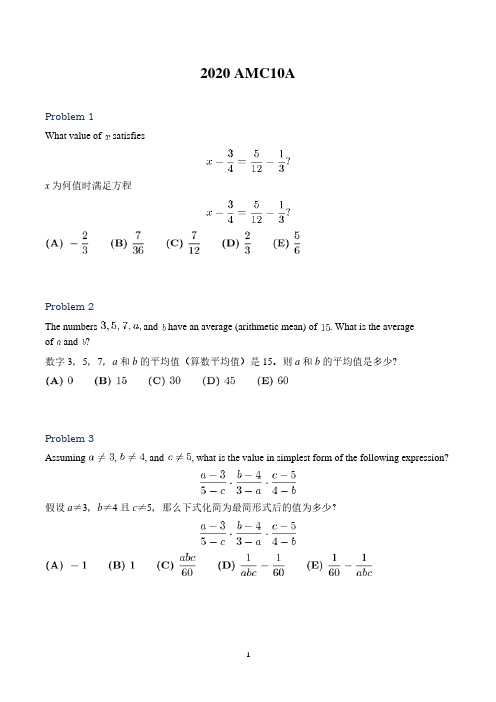
2020 AMC10AProblem 1What value of satisfiesx为何值时满足方程Problem 2The numbers and have an average (arithmetic mean) of . What is the averageof and ?数字3,5,7,a和b的平均值(算数平均值)是15。
则a和b的平均值是多少?Problem 3Assuming , , and , what is the value in simplest form of the following expression?假设a≠3,b≠4且c≠5,那么下式化简为最简形式后的值为多少?A driver travels for hours at miles per hour, during which her car gets miles per gallon of gasoline. She is paid per mile, and her only expense is gasoline at per gallon. What is her net rate of pay, in dollars per hour, after this expense?一位司机以60英里每小时的速度开了2小时,在此期间她的车每消耗1加仑的汽油可以开30英里。
她开1英里的收入是0.5美元,并且她的唯一支出是汽油支出,为每加仑2美元。
扣除掉此支出后,她的单位时间净收入是每小时多少美元?Problem 5What is the sum of all real numbers for which满足方程的所有实数x的值之和为多少?Problem 6How many -digit positive integers (that is, integers between and , inclusive) having onlyeven digits are divisible by有多少个各个数位上数字为偶数的4位正整数(即1000和9999之间的整数,包含1000和9999)能被5整除?Problem 7The integers from to inclusive, can be arranged to form a -by- square in which thesum of the numbers in each row, the sum of the numbers in each column, and the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum? 从-10到14(包含-10和14)之间的25个整数可以排列形成一个5x5的正方形,满足正方形的每一行,每一列以及每个主对角线的数字之和都相等。
美国数学竞赛2020年真题AMC10 A(真题加详解)
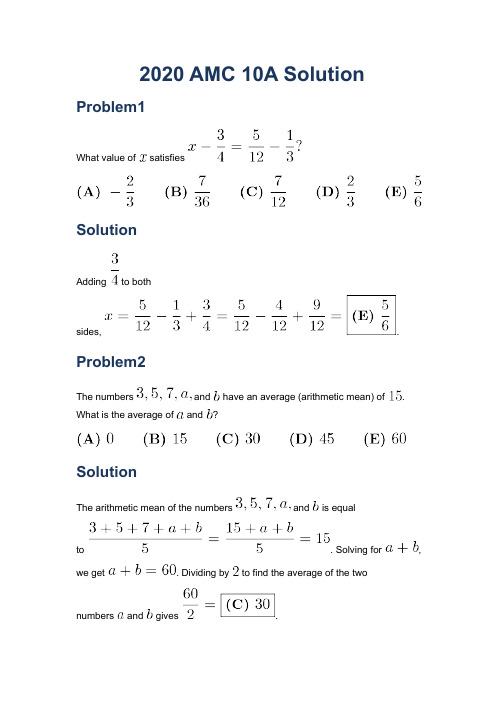
2020 AMC 10A Solution Problem1What value of satisfiesSolutionAdding to bothsides, . Problem2The numbers and have an average (arithmetic mean) of . What is the average of and ?SolutionThe arithmetic mean of the numbers and is equalto . Solving for , we get . Dividing by to find the average of the twonumbers and gives .Problem3Assuming , , and , what is the value in simplest form of the following expression?SolutionNote that is times .Likewise, is times and is times . Therefore, the product of the given fractionequals .Problem4A driver travels for hours at miles per hour, during which her cargets miles per gallon of gasoline. She is paid per mile, and her onlyexpense is gasoline at per gallon. What is her net rate of pay, in dollars per hour, after this expense?SolutionSince the driver travels 60 miles per hour and each hour she uses 2 gallons of gasoline, she spends $4 per hour on gas. If she gets $0.50 per mile, then she gets $30 per hour of driving. Subtracting the gas cost, her net rate of pay perhour is .Problem5What is the sum of all real numbers for whichSolution 1Split the equation into two cases, where the value inside the absolute value is positive and nonpositive.Case 1:The equation yields , which is equalto . Therefore, the two values for the positive caseis and .Case 2:Similarly, taking the nonpositive case for the value inside the absolute value notation yields . Factoring and simplifyinggives , so the only value for this case is .Summing all the values results in . Solution 2We have theequations and .Notice that the second is a perfect square with a double root at , and the first has real roots. By Vieta's, the sum of the roots of the first equationis .Problem6How many -digit positive integers (that is, integers between and , inclusive) having only even digits are divisible bySolutionThe ones digit, for all numbers divisible by 5, must be either or . However, from the restriction in the problem, it must be even, giving us exactly one choice () for this digit. For the middle two digits, we may choose any even integerfrom , meaning that we have total options. For the first digit, we follow similar intuition but realize that it cannot be , hence giving us 4 possibilities. Therefore, using the multiplication rule, weget . ~ciceroniiProblem7The integers from to inclusive, can be arranged to form a -by-square in which the sum of the numbers in each row, the sum of the numbers in each column, and the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum?SolutionWithout loss of generality, consider the five rows in the square. Each row must have the same sum of numbers, meaning that the sum of all the numbers in the square divided by is the total value per row. The sum of the integersis , and the common sum is .Solution 2Take the sum of the middle 5 values of the set (they will turn out to be the mean of each row). We get as our answer. ~BaolanProblem8What is the value ofSolution 1Split the even numbers and the odd numbers apart. If we group every 2 evennumbers together and add them, we get a total of . Summing the odd numbers is equivalent to summing the first 100 odd numbers, which is equal to . Adding these two, we obtain the answerof .Solution 2 (bashy)We can break this entire sum down into integer bits, in which the sum is , where is the first integer in this bit. We can find that the first sum of every sequence is , which we plug in for the bits in the entire sequenceis , so then we can plug it into the first term of every sequence equation we gotabove , and so the sum of every bit is , and we only found the value of , the sum of the sequenceis .-middletonkidsSolution 3Another solution involves adding everything and subtracting out what is not needed. The first step involvessolving. To do this, we can simply multiply and and divide by to getus . The next step involves subtracting out the numbers with minus signs. We actually have to do this twice, because we need to take out the numbers we weren’t supposed to add and then subtract them from the problem. Then, we can see that from to , incrementing by , there are numbers that we have to subtract. To do this we can do times divided by , and then we can multiply by , because we are counting by fours, not ones. Our answer will be , but remember, we have to do this twice. Once we do that,we will get . Finally, we just have to do , and our answer is .—Solution 4In this solution, we group every 4 terms. Our groups shouldbe: , ,, .... We add them together to get this expression: . This can be rewrittenas . We add this toget . ~BaolanSolution 5We can split up this long sum into groups of four integers. Finding the first few sums, we have that , , and . Notice that this is an increasing arithmetic sequence, with a common difference of . We can find the sum of the arithmetic sequence by finding the average of the first and last terms, and then multiplying by the number of terms in the sequence. The first term is , or , the last term is , or , and thereare or terms. So, we have that the sum of the sequenceis , or . ~Arctic_BunnySolution 3Taking the average of the first and last terms, and , we have that the mean of the set is . There are 5 values in each row, column or diagonal, so thevalue of the common sum is , or . ~Arctic_Bunny, edited by KINGLOGICProblem9A single bench section at a school event can hold either adults or children. When bench sections are connected end to end, an equal number of adults and children seated together will occupy all the bench space. What is the least possible positive integer value ofSolutionThe least common multiple of and is . Therefore, there mustbe adults and children. The total number of benchesis .Solution 2This is similar to Solution 1, with the same basic idea, but we don't need to calculate the LCM. Since both and are prime, their LCM must be theirproduct. So the answer would be . ~Baolan Problem10Seven cubes, whose volumes are , , , , , , and cubic units, are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top. Except for the bottom cube, the bottom face of each cube lies completely on top of the cube below it. What is the total surface area of the tower (including the bottom) in square units?Solution 1The volume of each cube follows the pattern of ascending, for isbetween and .We see that the total surface area can be comprised of three parts: the sides of the cubes, the tops of the cubes, and the bottom of the cube (which is just ). The sides areas can be measured as thesum , giving us . Structurally, if we examine the tower from the top, we see that it really just forms a square of area . Therefore, wecan say that the total surface area is . Alternatively, for the area of the tops, we could have found thesum , giving us as well.~ciceroniiSolution 2It can quickly be seen that the side lengths of the cubes are the integers from 1 to 7, inclusive.First, we will calculate the total surface area of the cubes, ignoring overlap. This valueis. Then, we need to subtract out the overlapped parts of the cubes. Between each consecutive pair of cubes, one of the smaller cube's faces is completely covered, along with an equal area of one of the larger cube's faces. The total area of theoverlapped parts of the cubes is thus equal to . Subtracting the overlapped surface area from the total surface area, weget . ~emerald_blockSolution 3 (a bit more tedious than others)It can be seen that the side lengths of the cubes using cube roots are all integers from to , inclusive.Only the cubes with side length and have faces in the surface area and the rest have . Also, since thecubes are stacked, we have to find the difference betweeneach and side length as ranges from to.We then come up withthis:.We then add all of this and get .Problem 11What is the median of the following list of numbersSolution 1We can see that is less than 2020. Therefore, there are ofthe numbers after . Also, there are numbers that are under and equal to . Since is equal to , it, with the other squares, willshift our median's placement up . We can find that the median of the whole set is , and gives us . Our answeris .~aryamSolution 2As we are trying to find the median of a -term set, we must find the average of the th and st terms.Since is slightly greater than , we know thatthe perfect squares through are less than , and the rest aregreater. Thus, from the number to the number , thereare terms. Since is less than and less than , we will only need to consider theperfect square terms going down from the th term, , after going down terms. Since the th and st terms areonly and terms away from the th term, we can simplysubtract from and from to get the two terms, whichare and . Averaging the two, weget ~emerald_blockSolution 3We want to know the th term and the th term to get the median. We know thatSo numbers are in between to .So the sum of and will result in , which means that is the th number.Also, notice that , which is larger than .Then the th term will be , and similarlythe th term will be .Solving for the median of the two numbers, we getProblem12Triangle is isoceles with .Medians and are perpendicular to each other,and . What is the area ofSolution 1Since quadrilateral has perpendicular diagonals, its area can be found as half of the product of the length of the diagonals. Also notethat has the area of triangle by similarity,so Thus,Solution 2 (Trapezoid)We know that , and since the ratios of its sidesare , the ratio of of their areas is .If is the area of , then trapezoid is the area of .Let's call the intersection of and . Let .Then . Since , and are heights of triangles and , respectively. Both of these triangles have base .Area ofArea ofAdding these two gives us the area of trapezoid , whichis .This is of the triangle, so the area of the triangleis ~quacker88, diagram by programjames1 Solution 3 (Medians)Draw median .Since we know that all medians of a triangle intersect at the incenter, we know that passes through point . We also know that medians of a triangle divide each other into segments of ratio . Knowing this, we can seethat , and since the two segments sumto , and are and , respectively.Finally knowing that the medians divide the triangle into sections of equal area, finding the area of is enough. .The area of . Multiplying this by givesus~quacker88Solution 4 (Triangles)We knowthat , , so .As , we can seethat and with a side ratio of .So , .With that, we can see that , and the area oftrapezoid is 72.As said in solution 1, .-QuadraticFunctions, solution 1 by ???Solution 5 (Only Pythagorean Theorem)Let be the height. Since medians divide each other into a ratio, and the medians have length 12, wehave and . From righttriangle ,so . Since is a median, . From righttriangle ,which implies . Bysymmetry .Applying the Pythagorean Theorem to righttriangle gives,so . Then the areaof isSolution 6 (Drawing)(NOT recommended) Transfer the given diagram, which happens to be to scale, onto a piece of a graph paper. Counting the boxes should give a reliable result since the answer choices are relatively far apart. -LingjunSolution 7Given a triangle with perpendicular medians with lengths and , the area will be .Solution 8 (Fastest)Connect the line segment and it's easy to seequadrilateral has an area of the product of its diagonals dividedby which is . Now, solving for triangle could be an option, but the drawing shows the area of will be less than the quadrilateral meaning the the area of is less than but greater than , leaving onlyone possible answer choice, .Problem 13A frog sitting at the point begins a sequence of jumps, where each jump is parallel to one of the coordinate axes and has length , and the direction of each jump (up, down, right, or left) is chosen independently at random. The sequence ends when the frog reaches a side of the square withvertices and . What is the probability that thesequence of jumps ends on a vertical side of the squareSolutionDrawing out the square, it's easy to see that if the frog goes to the left, it will immediately hit a vertical end of the square. Therefore, the probability of thishappening is . If the frog goes to the right, it will be in the center ofthe square at , and by symmetry (since the frog is equidistant from all sides of the square), the chance it will hit a vertical side of a square is . The probability of this happening is .If the frog goes either up or down, it will hit a line of symmetry along the corner it is closest to and furthest to, and again, is equidistant relating to the two closer sides and also equidistant relating the two further sides. The probability for it tohit a vertical wall is . Because there's a chance of the frog going up and down, the total probability for this case is and summing up all thecases,Solution 2Let's say we have our four by four grid and we work this out by casework. A is where the frog is, while B and C are possible locations for his second jump, while O is everything else. If we land on a C, we have reached the vertical side. However, if we land on a B, we can see that there is an equal chance of reaching the horizontal or vertical side, since we are symmetrically between them. So we have the probability of landing on a C is 1/4, while B is 3/4. Since C means that we have "succeeded", while B means that we have a half chance, wecompute .We get , or-yeskaySolution 3If the frog is on one of the 2 diagonals, the chance of landing on vertical or horizontal each becomes . Since it starts on , there is a chance (up,down, or right) it will reach a diagonal on the first jump and chance (left) it will reach the vertical side. The probablity of landing on a verticalis - Lingjun.Solution 4 (Complete States)Let denote the probability of the frog's sequence of jumps ends with ithitting a vertical edge when it is at . Note that by reflective symmetry over the line .Similarly, , and .Now we create equations for the probabilities at each of these points/states by considering the probability of going either up, down, left, or right from thatpoint:We have a system of equationsin variables, so we can solve for each of these probabilities. Plugging the second equation into the fourth equationgivesPlugging in the third equation into thisgivesNext, plugging in the second and third equation into the first equationyieldsNow plugging in (*) into this, wegetProblem14Real numbers and satisfy and . What is the value ofSolutionContinuing tocombineFrom the givens, it can be concluded that .Also, This meansthat . Substituting this informationinto , wehave . ~PCChess Solution 2As above, we need to calculate . Note that are the roots of andso and .Thuswhere and as in the previous solution. Thus the answer is .Solution 3Note that Now, we only need to find the values of andRecall that andthat We are able to solve thesecond equation, and doing so gets us Plugging this into the first equation, we getIn order to find the value of we find a common denominator so that we can add them together. This getsus Recallingthat and solving this equation, weget Plugging this into the first equation, wegetSolving the original equation, weget ~emerald_blockSolution 4 (Bashing)This is basically bashing using Vieta's formulas to find and (which I highly do not recommend, I only wrote this solution for fun).We use Vieta's to find a quadratic relating and . We set and to be the roots of the quadratic (because , and ). We can solve the quadratic to get theroots and . and are "interchangeable", meaning that it doesn't matter which solution or is, because it'll return the same result when plugged in. So we plug in for and andget as our answer.~BaolanSolution 5 (Bashing Part 2)This usually wouldn't work for most problems like this, but we're lucky that we can quickly expand and factor this expression in this question.We first change the original expression to ,because . This is equalto. We can factor andreduce to. Now our expression is just . Wefactor to get . So the answer would be .Solution 6 (Complete Binomial Theorem)We first simplify the expression to Then, we can solve for and given the system of equations in the problem.Since we can substitute for . Thus, this becomes theequation Multiplying both sides by , weobtain or By the quadratic formula we obtain . We also easily find thatgiven , equals the conjugate of . Thus, plugging our valuesin for and , our expression equalsBy the binomial theorem, we observe that every second terms of theexpansions and will cancel out (since a positive plus a negative of the same absolute value equals zero). We also observe that the other terms notcanceling out are doubled when summing the expansions of . Thus, our expression equals whichequals which equals .Problem15A positive integer divisor of is chosen at random. The probability that thedivisor chosen is a perfect square can be expressed as , where and are relatively prime positive integers. What is ?SolutionThe prime factorization of is . This yields a totalof divisors of In order to produce a perfect square divisor, there must be an even exponent for each number in the prime factorization. Note that and can not be in the prime factorization of a perfect square because there is only one of each in Thus, thereare perfect squares. (For , you can have , , , , , or 0 s, etc.) The probability that the divisor chosen is a perfect squareisProblem16A point is chosen at random within the square in the coordinate plane whose vertices are and . Theprobability that the point is within units of a lattice point is . (A point is a lattice point if and are both integers.) What is to the nearest tenthSolution 1DiagramDiagram by MathandSki Using AsymptoteNote: The diagram represents each unit square of thegiven square.SolutionWe consider an individual one-by-one block.If we draw a quarter of a circle from each corner (where the lattice points are located), each with radius , the area covered by the circles should be . Because of this, and the fact that there are four circles, we writeSolving for , we obtain , where with , we get , and from here, we simplify and seethat ~CrypthesTo be more rigorous, note that since if thenclearly the probability is greater than . This would make sure the above solution works, as if there is overlap with thequartercircles.Solution 2As in the previous solution, we obtain the equation , which simplifies to . Since is slightly more than , is slightly less than . We notice that is slightly morethan , so is roughly ~emerald_blockSolution 3 (Estimating)As above, we find that we need to estimate .Note that we can approximate andso .And so our answer is .Problem 17Define How many integers are there such that ?Solution 1Notice that is a product of many integers. We either need one factor to be 0 or an odd number of negative factors.Case 1: There are 100 integers for whichCase 2: For there to be an odd number of negative factors, must be between an odd number squared and an even number squared. This means that thereare total possible values of . Simplifying, thereare possible numbers.Summing, there are total possible values of . ~PCChess Solution 2Notice that is nonpositive when isbetween and , and , and (inclusive), whichmeans that the amount of valuesequals.This reducesto~ZericSolution 3 (end behavior)We know that is a -degree function with a positive leading coefficient. Thatis, .Since the degree of is even, its end behaviors match. And since theleading coefficient is positive, we know that both ends approach as goes in either direction.So the first time is going to be negative is when it intersects the -axis atan -intercept and it's going to dip below. This happens at , which is the smallest intercept.However, when it hits the next intercept, it's going to go back up again into positive territory, we know this happens at . And when it hits , it's going to dip back into negative territory. Clearly, this is going to continue to snake around the intercepts until .To get the amount of integers below and/or on the -axis, we simply need to count the integers. For example, the amount of integers in betweenthe interval we got earlier, we subtract and addone. integers, so there are four integers in this interval that produce a negative result.Doing this with all of the other intervals, we have. Proceed with Solution 2. ~quacker88Problem18Let be an ordered quadruple of not necessarily distinct integers, each one of them in the set For how many such quadruples is it truethat is odd? (For example, is one such quadruple, because is odd.)SolutionSolution 1 (Parity)In order for to be odd, consider parity. We must have (even)-(odd) or (odd)-(even). There are ways to pick numbers to obtain an even product. There are ways to obtain an odd product. Therefore, the total amount of ways to make oddis .-MidnightSolution 2 (Basically Solution 1 but more in depth)Consider parity. We need exactly one term to be odd, one term to be even. Because of symmetry, we can set to be odd and to be even, then multiply by If is odd, both and must be odd, therefore thereare possibilities for Consider Let us say that is even. Then there are possibilities for However, can be odd, in which case we have more possibilities for Thus there are ways forus to choose and ways for us to choose Therefore, also consideringsymmetry, we have total values ofSolution 3 (Complementary Counting)There are 4 ways to choose any number independently and 2 ways to choose any odd number independently. To get an even products, wecount: , which is . The number of ways to get an odd product can be counted like so: , which is , or . So, for oneproduct to be odd the other to be even: (order matters). ~ Anonymous and Arctic_BunnySolution 4 (Solution 3 but more in depth)We use complementary counting: If the difference is even, then we can subtract those cases. There are a total of cases.For an even difference, we have (even)-(even) or (odd-odd).From Solution 3:"There are 4 ways to choose any number independently and 2 ways to choose any odd number independently. even products:(number)*(number)-(odd)*(odd): . odd products: (odd)*(odd): ."With this, we easily calculate . Problem19As shown in the figure below, a regular dodecahedron (the polyhedron consisting of congruent regular pentagonal faces) floats in space with two horizontal faces. Note that there is a ring of five slanted faces adjacent to the top face, and a ring of five slanted faces adjacent to the bottom face. How many ways are there to move from the top face to the bottom face via a sequence of adjacent faces so that each face is visited at most once and moves are not permitted from the bottom ring to the top ring?DiagramSolution 1Since we start at the top face and end at the bottom face without moving from the lower ring to the upper ring or revisiting a face, our journey must consist of the top face, a series of faces in the upper ring, a series of faces in the lower ring, and the bottom face, in that order.We have choices for which face we visit first on the top ring. From there, we have choices for how far around the top ring we go before movingdown: or faces around clockwise, or faces aroundcounterclockwise, or immediately going down to the lower ring without visiting any other faces in the upper ring.We then have choices for which lower ring face to visit first (since every upper-ring face is adjacent to exactly lower-ring faces) and then once again choices for how to travel around the lower ring. We then proceed to the bottom face, completing the trip.Multiplying together all the numbers of choices we have, weget .Solution 2Swap the faces as vertices and the vertices as faces. Then, this problem is the same as 2016 AIME I #3which had an answerof .Problem20Quadrilateral satisfiesand Diagonals and intersect at point and What is the area of quadrilateralSolution 1 (Just Drop An Altitude)It's crucial to draw a good diagram for this one.Since and , we get . Now weneed to find to get the area of the whole quadrilateral. Drop an altitudefrom to and call the point of intersection . Let .Since , then . By dropping this altitude, we can also see two similar triangles, and .Since is , and , we get that . Now, if we redraw another diagram just of , we getthat . Now expanding, simplifying, and dividing by the GCF, we get . This factorsto . Since lengths cannot be negative, .Since , .So(I'm very sorry if you're a visual learner but now you have a diagram by ciceronii) ~ Solution by Ultraman~ Diagram by ciceroniiSolution 2 (Pro Guessing Strats)We know that the big triangle has area 300. Use the answer choices which would mean that the area of the little triangle is a multiple of 10. Thus the product of thelegs is a multiple of 20. Guess that the legs are equal to and , and because the hypotenuse is 20 we get . Testing small numbers, we get that when and , is indeed a square. The area of the triangle is thus 60, so the answer is .~tigershark22 ~(edited by HappyHuman)Solution 3 (coordinates)Let the pointsbe , , , ,and , respectively. Since lies on line , we know that . Furthermore, since , lies on the circle with diameter , so . Solving for and with these equations, we get the solutions and . We immediately discardthe solution as should be negative. Thus, we concludethat.Solution 4 (Trigonometry)Let and Using Law of Sineson we get and LoSon yieldsDivide the two toget Now,and solve the quadratic, taking the positive solution (C is acute) toget Soif then and By Pythagorean Theorem, and the answeris(This solution is incomplete, can someone complete it please-Lingjun) ok Latex edited by kc5170We could use the famous m-n rule in trigonometry in triangle ABC with Point E [Unable to write it here.Could anybody write the expression] We will find that BD is angle bisector of triangle ABC(because we will get tan (x)=1) Therefore by converse of angle bisector theorem AB:BC = 1:3. By using phythagorean theorem we have values of AB and AC. AB.AC = 120. Adding area of ABC and ACD Answer••360Problem21There exists a unique strictly increasing sequence of nonnegativeintegers suchthat What isSolution 1First, substitute with . Then, the given equationbecomes . Now consider only . Thisequals . Notethat equals , since the sum of a geometricsequence is . Thus, we can see that forms the sum of 17 different powers of 2. Applying the same method to eachof , , ... , , we can see that each of the pairs forms the sum of 17 different powers of 2. This gives us . But we must count also the term. Thus, Our answeris .~seanyoon777Solution 2(This is similar to solution 1) Let . Then, . The LHS canbe rewrittenas。
2012年-AMC10-A卷 中文翻译及答案

13. 将 1,2,3,4,5 依某种顺序排列,对于这种排列的逐次平均数的计算方式如 下:先找出最前面两数的平均数;再求此平均数与第三数的平均数:再求第二次得 到的平均数与第四数的平均数;最后再求第三次得到的平均数与第五数的平均数; 如此最终所得到的平均数称为此排列的逐次平均数。试问 1,2,3,4,5 所有可能 的排列中,最大的逐次平均数与最小的逐次平均数的差为多少? (A) 31 16 (B) 2 (C) 17 8 (D) 3 (E) 65 16
A C B
(A)
1 6
(B)
1 5
(C)
2 9
(D)
1 3
(E)
2 4
16. 三名赛跑选手同时、同向、从同一起跑点开始在一个 500 公尺长的圆形跑道上 赛跑,三位选手的速率分别为 4.4 公尺/秒、4.8 公尺/秒及 5 公尺/秒,且他们都 维持各自固定的速率跑,则从开始到他们下一次都碰在一起共花了几秒钟? (A) 1000 (B) 1250 (C) 2500 (D) 5000 (E) 10000
20. 将一个边长为 3 的大正方形画分成 9 个边长为 1 的小正方形格子, 并随意将每 一个格子涂成白色或黑色。以大正方形中心为旋转中心顺时针转 90后,除将原来 位置是黑色但旋转后变成白色的格子再涂成黑色外,其它的格子颜色维持不变,此 时大正方形的所有格子都是黑色的机率是多少? (A) 49 512 (B) 7 64 (C) 121 1024 (D) 81 512 (E) 9 32
14. 小贝制作了一块非典型的正方形西洋棋盘,每边有 31 个小正方形。若此棋盘 的四个角落都是黑色的小正方形,且各行各列都是黑、红相间的小正方形,则此棋 盘上总共有多个黑色的小正方形? (A) 480 (B) 481 (C) 482 (D) 483 (E) 484
AMC10美国数学竞赛A卷附中文翻译和答案

AMC10 美国数学竞赛 A 卷附中文翻译和答案简介AMC10 是美国的一项全国性数学竞赛,主要面向高中学生。
此文档提供了 AMC10 美国数学竞赛 A 卷的问题、答案及中文翻译,帮助考生更好地理解和准备竞赛。
问题和答案问题 1让我们从一个整数开始,每一步都按照以下规则进行操作:如果当前的数是偶数,将它除以 2;如果当前的数是奇数,将它乘以 3 并加 1。
通过这样的操作,我们可以生成一个数列,例如,从 9 开始的数列如下所示:9,28,14,7,22,11,34,...。
显然,这个数列最终会包含两个连续的 1。
以下哪个数开始操作后会生成包含两个连续的 1?$\\textbf{(A)}\\ 111 \\qquad \\textbf{(B)}\\ 120 \\qquad \\textbf{(C)}\\ 125 \\qquad \\textbf{(D)}\\ 130 \\qquad\\textbf{(E)}\\ 139$$\\textbf{(D)}\\ 130$问题 2如果 $2^x \\times 5^y = 5000000$,那么x+x的值是多少?$\\textbf{(A)}\\ 6 \\qquad \\textbf{(B)}\\ 7 \\qquad\\textbf{(C)}\\ 10 \\qquad \\textbf{(D)}\\ 12 \\qquad\\textbf{(E)}\\ 15$答案 2$\\textbf{(C)}\\ 10$问题 3数轴上三个数x、x、x的平均值是 6。
给定x−x=8,x−x=12,那么x的值是多少?$\\textbf{(A)}\\ -10 \\qquad \\textbf{(B)}\\ -6 \\qquad \\textbf{(C)}\\ 0 \\qquad \\textbf{(D)}\\ 6 \\qquad\\textbf{(E)}\\ 10$答案 3$\\textbf{(B)}\\ -6$某菜市场的一个南瓜重 100 磅,其中 99% 的重量是水分。
美国数学测评AMC10A-2018(中英双语)

2018 AMC10A Problems1. What is the value of上述表达式的结果是多少?2. Liliane has 50% more soda than Jacqueline, and Alice has 25% more soda than Jacqueline. Whatis the relationship between the amounts of soda that Liliane and Alice have?(A)Liliane has 20% more soda than Alice.(B)Liliane has 25% more soda than Alice.(C)Liliane has 45% more soda than Alice.(D)Liliane has 75% more soda than Alice.(E)Liliane has 100% more soda than Alice.已知 Liliane 拥有汽水的量比Jacqueline 多50%,Alice 拥有汽水的量比Jacqueline 多25%。
请问 Liliane 和Alice拥有汽水量的关系是什么?(A)Liliane 的汽水量比Alice 多20%。
(B)Liliane 的汽水量比Alice 多25%。
(C)Liliane 的汽水量比Alice 多45%。
(D)Liliane 的汽水量比Alice 多75%。
(E)Liliane 的汽水量比Alice 多100%。
3. A unit of blood expires after 10! = 10 ·9 ·8 ···1 seconds. Yasin donates a unit of blood at noon on January 1. On what day does his unit of blood expire?(A) January 2(B) January 12 (C) January 22(D) February 11 (E) February 12一个单位的血液会在10! = 10 ·9 ·8 ···1秒后失效。
2020年美国数学竞赛(AMC10A)的试题与解答

2020年美国数学竞赛(AMC10A)的试题与解答广东省广州市华南师范大学(510631)李湖南1.What value of x satisfies x−34=512−13?A.−23B.736C.712D.23E.56译文方程x−34=512−13的解x是多少?解化简可得x=34+112=56,故(E)正确.2.The numbers3,5,7,a,and b have an average(arithmetic mean)of15.What is the average of a and b?A.0B.15C.30D.45E.60译文数字3,5,7,a和b的平均值(算术平均值)是15.则a和b的平均值是多少?解依题意有3+5+7+a+b=15×5,则a+b=60,平均值为30,故(C)正确.3.Assuming a=3,b=4and c=5,what is the value insimplest form of the following expression a−35−c·b−43−a·c−54−bA.−1B.1C.abc60D.1abc−160E.160−1abc译文设a=3,b=4,c=5,则表达式a−35−c·b−43−a·c−54−b的最简形式是什么?解约分即得a−35−c·b−43−a·c−54−b=−1,故(A)正确.4.A driver travels for2hours at60miles per hour,during which her car gets30miles per gallon of gasoline.She is paid $0.50per mile,and her only expense is gasoline at$2.00per gal-lon.What is her net rate of pay,in dollars per hour,after this expense?A.20B.22C.24D.25E.26译文一位司机以60英里/小时的速度驾车2小时,她的车每跑30英里需要消耗1加仑汽油.她能获得0.50美元/英里的报酬,唯一的花费就是2美元/加仑的汽油.问她每小时除去消耗之后的净收益是多少美元?解1个小时她能跑60英里,获得60×0.50=30美元,汽油费为60÷30×2=4美元,故净收益为26美元,(E)正确.5.What is the sum of all real numbers x for whichx2−12x+34=2?A.12B.15C.18D.21E.25译文满足方程x2−12x+34=2的所有实数x之和是多少?解分别解方程x2−12x+34=2和x2−12x+34=−2,可得x1,2=4,8和x3,4=6,故和为18,(C)正确.6.How many4-digit positive integers(that is,integers be-tween1000and9999,inclusive)having only even digits are di-visible by5?A.80B.100C.125D.200E.500译文有多少个四位的正整数(也就是在1000和9999之间的整数)能被5整除且所有数字均为偶数?解依题意,符合条件的四位数的个位数只能是0,十位数和百位数可以是0,2,4,6,8,千位数只能是2,4,6,8,共有1×5×5×4=100种选择,故(B)正确.7.The25integers from−10to14,inclusive,can be ar-ranged to form a5-by-5square in which the sum of the numbers in each row,the sum of the numbers in each column,the sum of the numbers along each of the main diagonals are all the same. What is the value of this common sum?A.2B.5C.10D.25E.50译文将25个整数分别是从−10到14,放入5×5的格子中,使得格子里的每行、每列和两条对角线的数字和均相等.问这个数字和是多少?解这是一个5阶幻方问题,25个数字之和是(−10)+(−9)+···+13+14=50,分别放入5行,故每行的数字和是10,(C)正确.8.What is the value of1+2+3−4+5+6+7−8+···+197+198+199−200?A.9800B.9900C.10000D.10100E.10200译文1+2+3−4+5+6+7−8+···+197+198+199−200的值是多少?解原式=1+(2+3−4)+5+(6+7−8)+···+197+(198+199−200)=2×(1+5+···+197)=2×(1+197)×502=9900,故(B)正确.9.A single bench section at a school event can hold either7adults or11children.When N bench sections are connected end to end,an equal number of adults and children seated together will occupy all the bench space.What is the least possible posi-tive integer value of N?A.9B.18C.27D.36E.77译文在某学校的活动中,一条长凳可以坐7个成人或者11个儿童.当N条长凳首尾相接的时候,刚好坐满了相同数量的成人和儿童.问N的最小正整数值是多少?解设有x条长凳坐了儿童,则有N−x条长凳坐了成人,依题意有11x=7(N−x),因而xN−x=711⇒xN=718.故N min=18,(B)正确.10.Seven cubes,whose volumes are1,8,27,64,125, 216,and343cubic units,are stacked vertically to form a tower in which the volumes of the cubes decrease from bottom to top. Except for the bottom cube,the bottom face of each cube lies completely on top of the cube below it.What is the total surface area of the tower(including the bottom)in square units?A.644B.658C.664D.720E.749译文七个立方体,体积分别是1,8,27,64,125,216,343个立方单位,依次按照体积大小由底到顶垂直地堆积成一座塔.除了最底部的立方体,每个立方体的底面都完全被下面的立方体的顶面覆盖.问这座塔的表面积(包括底面)是多少个平方单位?解这七个数都是立方数,则这七个立方体的棱长分别是1,2,3,4,5,6,7,从而塔的侧面积为4×(12+22+...+72)= 560,而上、下底面积之和为2×72=98,共658,故(B)正确.11.What is the median of the following list of4040num-bers?1,2,3,...,2020,12,22,32,...,20202A.1974.5B.1975.5C.1976.5D.1977.5E.1978.5译文下列4040个数:1,2,3,...,2020,12,22,32,..., 20202的中位数是多少?解由于442=1936,452=2025,从而以上数列按递增排列的话,就成为:1,12,...,4,22,...,1936,442,...,1976,1977,...,2020,452,462, (20202)此时,1976成为第2020个数,所求中位数为1976+19772=1976.5,故(C)正确.12.Triangle∆AMC is isosceles with AM=AC.Me-dians MV and CU are perpendicular to each other,and MV=CU=12.What is the area of∆AMC?A.48B.72C.96D.144E.192译文等腰∆AMC中,AM=AC,中线MV和CU互相垂直,且MV=CU=12.则∆AMC的面积是多少?解如图示,设MV交UC于点E,过A作AD⊥MC于D,交UV于F,则AD是MC的中垂线.依题意,U,V分别是AM,AC的中点,则UV是∆AMC的中位线,且UM=V C=12AM,从而∆UMC =∆V CM(SSS),可得∠UCM=∠V MC=45◦,因此图1EM=EC,点E在AD上.于是∆UV E ∆CME,且均是等腰直角三角形,因而UECE=UVCM=12⇒CE=23CU=8,进而CD=DE=4√2,EF=12DE=2√2,MC=2DC=8√2;又∆AUV ∆AMC,得AFAD=UVMC=12⇒AD=2DF=12√2.故S∆AMC=12MC·AD=8√2×12√22=96,(C)正确.13.A frog sitting at the point(1,2)begins a sequence ofjumps,where each jump is parallel to one of the coordinate ax-es and has length1,and the direction of each jump(up,down,left,right)is chosen independently at random.The sequence endswhen the frog reaches a side of the square with vertices(0,0),(0,4),(4,0),and(4,4).What is the probability that the sequence ofjumps ends on a vertical side of the square?A.12B.58C.23D.34E.78译文一只青蛙坐在点(1,2)上,开始一系列的跳跃,每次跳跃都平行于坐标轴且长度为1,方向(上、下、左、右)是随机的且独立,当青蛙到达由点(0,0),(0,4),(4,0),(4,4)构成的正方形的一条边的时候,跳跃终止.问跳跃终止于正方形竖直的两条边上的概率是多少?解如图示,青蛙在点F 1处,它可以向四个方向跳跃,概率均为14,向左跳跃,立刻达成目标;向上、向右、向下分别跳跃到点A 1,C,A 3处,再通过其它跳跃达成目标.根据对称性,青蛙由点A 1,A 2,A 3,A4图2出发达成目标的概率是一样的,设为a ;青蛙由点B 1,B 2出发达成目标的概率是一样的,设为b ;青蛙由点F 1,F 2出发达成目标的概率是一样的,设为x ;青蛙由点C 出发达成目标的概率设为c .因此,P (青蛙由F 1出发达成目标)=P (青蛙向左)+P (青蛙向上)×P (青蛙由A 1出发达成目标)+P (青蛙向右)×P (青蛙由C 出发达成目标)+P (青蛙向下)×P (青蛙由A 3出发达成目标),即有x =14+a 2+c4;同理,可得方程组x =14+a 2+c 4a =14+x 4+b 4b =a 2+c 4c =x 2+b 2成立,解得x =58,a =12,b =38,c =12.故(B)正确.14.Real numbers x and y satisfies x +y =4and xy =−2.What is the value of x +x 3y 2+y 3x2+y ?A.360B.400C.420D.440E.480译文实数x,y 满足方程x +y =4和xy =−2.则x +x 3y 2+y 3x2+y 的值是多少?解依题意可得x 2+y 2=(x +y )2−2xy =20,x 3+y 3=(x +y )(x 2−xy +y 2)=88,原式=x +y 3x 2+y +x 3y 2=x 3+y 3x 2+x 3+y 3y 2=88(x 2+y 2)x 2y 2=440.故(D)正确.15.A positive integer divisor of 12!is chosen at random.The probability that the divisor is a perfect square can be ex-pressed as mn,where m and n are relatively prime positive in-tegers.What is m +n ?A.3B.5C.12D.18E.23译文随机选取12!的一个正整数因子,该因子是一个完全平方数的概率可以表示为mn,其中m,n 为互素的正整数.则m +n 是多少?解由于12!=210×35×52×7×11,则12!有11×6×3×2×2=792个正因子;设k 是12!的平方因子,则k =2a ×3b ×5c ×7d ×11i ,其中a 10,b 5,c 2,d 1,i 1,且a,b,c,d,i 均为非负偶数,即a =0,2,4,6,8,10,b =0,2,4,c =0,2,d =i =0,此时k 有6×3×2=36种选择.从而所求概率为36792=122.故m +n =23,(E)正确.16.A point is chosen at random within the square in the co-ordinate plane whose vertices are (0,0),(2020,0),(2020,2020),and (0,2020).The probability that the point lies within d unitsof a lattice point is 12.(A point (x,y )is a lattice point if x and yare both integers.)What is d to the nearest tenth?A.0.3B.0.4C.0.5D.0.6E.0.7译文坐标平面上有一个以(0,0),(2020,0),(2020,2020)和(0,2020)为顶点的正方形.在正方形内随机选择一个点,该点位于格点的d 个单位内的概率是12.(点(x,y )称为格点,若x 和y 均为整数.)图3则d 精确到十分位是多少?解如图示,以格点为圆心,d 为半径作一些圆,则正方形内的圆内部分就是符合条件的点集.因此,该点落在此区域的概率为P =该区域的面积正方形面积,即12=(20192+2019×2+1)πd 220202=πd 2,求得d =√12π≈0.4,故(B)正确.17.Define P (x )=(x −12)(x −22)···(x −1002).How many integers n are there such that P (n ) 0?A.4900B.4950C.5000D.5050E.5100译文定义P (x )=(x −12)(x −22)···(x −1002).则有多少个整数n 使得P (n ) 0?解解不等式P (n )=(n −12)(n −22)···(n −1002) 0,得12 n 22,32 n 42,···,992 n 1002,因此符合条件的n 有(22−12+1)+(42−32+1)+···+(1002−992+1)=2×(2+4+···+100)=5100个.故(E)正确.18.Let (a,b,c,d )be an ordered quadruple of not necessar-ily distinct integers,each one of them in the set {0,1,2,3}.For how many such quadruples is it true that ad −bc is odd?(For ex-ample,(0,3,1,1)is one such quadruple,because 0·1−3·1=−3is odd.)A.48B.64C.96D.128E.192译文设(a,b,c,d)是一个四元数,其中a,b,c,d∈{0,1,2,3}且可以相同.则有多少个这样的四元数使得ad−bc是奇数?(例如,(0,3,1,1)就是一个符合条件的四元数,因为0·1−3·1=−3是奇数.)解要使得ad−bc是奇数,ad和bc必一奇一偶,分两种情况:(1)ad是奇数,bc是偶数:此时a,d∈{1,3},b,c可以是一奇一偶、一偶一奇、两个偶数,共有2×2×(2×2×3)=48种选择;(2)ad是偶数,bc是奇数:同理可得48种选择.故共有96个,(C)正确.19.As shown in the figure below,a regular dodecahedron (the polyhedron consisting of12congruent regular pentagonal faces)floats in space with two horizontal faces.Note that there is a ring of five slanted faces adjacent to the top face,and a ring of five slanted faces adjacent to the bottom face.How many ways are there to move from the top face to the bottom face via a se-quence of adjacent faces so that each face is visited at most once and moves are not permitted from the bottom ring to the top ring?A.125B.250C.405D.640E.810译文如下图左所示,一个正十二面体(由12个完全相同的正五边形面组成的多面体)放置在两个水平面的空间中.注意到顶面附近有一个由五个斜面组成的圆环,底面附近有一个由五个斜面组成的圆环.问有多少种方法可以通过一系列相邻面从顶面移动到底面,使得每个面最多经过一次,并且不允许从底环移动到顶环?图4图5解将该图简化成平面图:顶面为点T,底面为点B,顶环依次为点T1,T2,T3,T4,T5,底环依次为点B1,B2,B3,B4,B5,相邻的两个面用线段连接,如上图右所示.则原问题相当于:有多少条从点T出发,经过顶环,再经过底环,最后到达点B的不重复路径?(1)点T到顶环,有5种选择,即T1,T2,T3,T4,T5;(2)不妨设先到T1,又有9种选择到达底环,分别是:T1→,T1→T2→,T1→T2→T3→,T1→T2→T3→T4→,T1→T2→T3→T4→T5→,T1→T5→,T1→T5→T4→,T1→T5→T4→T3→,T1→T5→T4→T3→T2→;(3)每一个顶环上的点有2种方式到达底环,如T1→B1或T1→B5;(4)以到达B1为例,有以下9条路径到达点B,分别是:B1→B,B1→B2→B,B1→B2→B3→B,B1→B2→B3→B4→B,B1→B2→B3→B4→B5→B,B1→B5→B,B1→B5→B4→B,B1→B5→B4→B3→B,B1→B5→B4→B3→B2→B.综上分析可得,共有5×9×2×9=810条路径,故(E)正确.20.Quadrilateral ABCD satisfies∠ABC=∠ACD=90◦,AC=20and CD=30.Diagonals AC and BD inter-sects at point E and AE=5.What is the area of QuadrilateralABCD?A.330B.340C.350D.360E.370译文四边形ABCD满足∠ABC=∠ACD=90◦,AC=20,CD=30.对角线AC和BD交于点E,且AE=5.求四边形ABCD的面积是多少?解如图示,以AC为直径作一个圆,交BD与点F,图6依题意可得EC=15,ED=√EC2+CD2=15√5.设BE=x,依据相交弦定理AE·EC=BE·EF,则得EF=75x,DF=15√5−75x,DB=15√5+x;再由切割线定理DC2=DF·DB,得900=(15√5−75x)·(15√5+x),解得x=3√5或x=−5√5(舍去).而S∆ACD=12×20×30=300,S∆ABCS∆ACD=BEED=15,可得S∆ABC=60,故S ABCD=360,(D)正确.21.There exists a unique strictly increasing sequence ofnonnegative integers a1<a2<···<a k such that2289+1217+1=2a1+2a2+···+2a k.What is k?A.117B.136C.137D.273E.306译文存在唯一严格递增的非负整数列a1<a2<···<a k使得2289+1217+1=2a1+2a2+···+2a k,则k是多少?解令217=x ,则2289+1217+1=x 17+1x +1=x 16−x 15+x 14−x 13+···+x 2−x +1,而x 16−x 15=2272−2255=2271+2270+···+2255,同理x14−x13=2238−2221=2237+2236+···+2221,···,x 2−x =234−217=233+232+···+217,从而2189+1217+1=20+(217+···+232+233)+···+(2255+···+2270+2271)共8×17+1=137项,故(C)正确.22.For how many positive integers n 1000is ⌊998n ⌋+⌊999n ⌋+⌊1000n⌋not divisible by 3?(recall that ⌊x ⌋is the great-est integer less than or equal to x .)A.22B.23C.24D.25E.26译文有多少个正整数n 1000使得⌊998n ⌋+⌊999n ⌋+⌊1000n ⌋不被3整除?(注意⌊x ⌋表示小于等于x 的最大整数)解当n 不是998,999或1000的因子时,易得⌊998n ⌋=⌊999n ⌋=⌊1000n ⌋,从而N =⌊998n ⌋+⌊999n ⌋+⌊1000n⌋能被3整除;由于998=2×499,999=33×37,1000=23×53,只有1和2两个公因子,共有4+8+16−2−1=25个不同的因子,分情况讨论:(1)公因子:n =1时,N =2997能被3整除;n =2时,N =1498不能被3整除;(2)998的非公因子(2个):⌊998n ⌋=⌊999n ⌋=⌊1000n ⌋,N 能被3整除;(3)999的非公因子:N =⌊998n ⌋+⌊999n ⌋+⌊1000n⌋=3·999n −1不能被3整除;(4)1000的非公因子:N =⌊998n ⌋+⌊999n ⌋+⌊1000n⌋=3·1000n−2不能被3整除.综上可得,符合条件的n 有25−3=22个,故(A)正确.23.Let T be the triangle in the coordinate plane with ver-tices (0,0),(4,0),and (0,3).Consider the following five isome-tries (rigid transformations)of the plane:rotation of 90◦,180◦,and 270◦counterclockwise around the origin,reflection across the x -axis,and reflection across the y -axis.How many of the 125sequences of three of these transformations (not necessarily dis-tinct)will return T to its original position?(For example,a 180◦rotation,followed by a reflection across the x -axis,followed bya reflection across the y -axis will return T to its original position,but a 90◦rotation,followed by a reflection across the x -axis,fol-lowed by another reflection across the x -axis will not return T to its original position.)A.12B.15C.17D.20E.25译文设T 是坐标平面上以(0,0),(4,0)和(0,3)为顶点的三角形.考虑以下五种平面上的等距变换(刚体变换):绕原点作90◦,180◦和270◦的逆时针旋转,关于x 轴或y 轴的反射.任选三种变换(不必不同)可以组成125种组合,有图7多少种组合将使得T 变回起始位置?(例如,一个关于y 轴的反射,接着一个关于x 轴的反射,再接着一个180◦的旋转,将会使得T 变回起始位置;但一个关于x 轴的反射,接着另一个关于x 轴的反射,再接着一个90◦的旋转,将不会使得T 变回起始位置.)解分两种情况:(1)全部由旋转组成:只要三次旋转的角度和为360◦或720◦即可满足要求,因此有90◦+90◦+180◦,90◦+180◦+90◦,180◦+90◦+90◦,270◦+270◦+180◦,270◦+180◦+270◦,180◦+270◦+270◦共6种组合;(2)由旋转和反射组合而成:有y 轴+x 轴+180◦,y 轴+180◦+x 轴,180◦+x 轴+y 轴,180◦+y 轴+x 轴,x 轴+180◦+y 轴,x 轴+y 轴+180◦,也是6种组合.故(A)正确.24.Let n be the least positive integer greater than 1000for which gcd (63,n +120)=21and gcd (n +63,120)=60.What is the sum of digits of n ?A.12B.15C.18D.21E.24译文设n 是大于1000的使gcd (63,n +120)=21,gcd (n +63,120)=60成立的最小正整数.则n 的数字和是多少?解由gcd (63,n +120)=21,可得n ≡6(mod 21);由gcd (n +63,120)=60,可得n ≡57(mod 60).联立解得n ≡237(mod 420),于是n =1077,1497,1917,···.当n =1077时,gcd (63,n +120)=63,不符;当n =1497时,gcd (n +63,120)=120,也不符;当n =1917时,验证后符合条件,此时数字和为18,故(C)正确.例谈伸缩变换在解高考题中的应用广东省佛山市高明区教师发展中心(528500)张文玲一、定义引出在高中数学选修4-4第一讲中,有如下定义:设点P (x,y )是平面直角坐标系中的任意一点,在变换φ: x ′=λx (λ>0)y ′=µy (µ>0)的作用下,点P (x,y )对应到点P ′(x ′,y ′),称φ为平面直角坐标系中的坐标伸缩变换,简称伸缩变换.在它的作用下,可以实现平面图形的伸缩.对于椭圆x 2a 2+y 2b 2=1(a >b >0),在变换φ:x ′=xy ′=my(简言之,横坐标不变,纵坐标拉伸,m 称为伸缩率)的作用下,变为圆x ′2+y ′2=a 2,其中m =ab[1].在此变换下,原来直角坐标系xOy 中的点A (x 1,y 1),B (x 2,y 2),C (x 3,y 3)对应变为直角坐标系x ′O ′y ′中的点A ′(x 1′,y 1′),B ′(x 2′,y 2′),C ′(x 3′,y 3′),且有如下结论:1⃝若点A,B,C 三点共线,则点A ′,B ′,C ′三点也共线[1].2⃝若A,B,C 三点共线且|AB |=λ|BC |(λ>0),则变换之后|A ′B ′|=λ|B ′C ′|(λ>0).故若点B 为线段AC 的中点,则点B ′为线段A ′C ′的中点[1].3⃝若直线AB 的斜率为k ,则直线A ′B ′的斜率为mk [1].故两条平行直线经变换后仍然平行.4⃝两封闭图形的面积之比在变换前后不变.在圆中有很多优美的性质,将椭圆伸缩变换为圆之后,就可以利用圆的性质来解决一些问题,简化了计算,使学生不再“望椭圆而生畏”.下面从圆的四个常见性质出发来解决一些涉及到椭圆的高考题目.二、应用举例(一)直径所对的圆周角是直角例1(2019年高考全国Ⅱ卷理科第21题)已知点A (−2,0),B (2,0),动点M (x,y )满足直线AM 与BM 的斜率之积为−12.记M 的轨迹为曲线C .25.Jason rolls three fair standard six-sided dice.Then he looks at the rolls and chooses a subset of the dice (possibly emp-ty,possibly all three dice)to reroll.After rerolling,he wins if and only if the sum of the numbers faces up on the three dice is exactly 7.Jason always plays to optimize his chances of winning.What is the probability that he chooses to reroll exactly two of the dice?A.736B.524C.29D.1772E.14译文詹森掷3颗标准、均匀的骰子,他看了结果之后会选择若干(可能是0,也可能是3)颗重掷.当3颗骰子正面朝上的数字和为7点的时候,他就赢了.詹森总是按照朝着他赢的最优策略去掷.问他刚好选择2颗骰子重掷的概率是多少?解掷1颗骰子得1,2,3,4,5,6点的概率均为16;掷2颗骰子得3点只有两种情况:12和21,概率为236,···;掷3颗骰子得7点有15种情况:115,151,511,124,142,214,241,412,421,133,313,331,223,232,322,概率为15216,···.经过计算,所有结果如下表所示:分类/概率/结果1234567掷1颗161616161616掷2颗136236336436536掷3颗1216321632161021615216因此,詹森要选择2颗骰子重掷,则上次掷的结果中,任意两颗骰子的数字和不能小于7点,否则他将选择重掷1颗骰子;且不能3颗骰子都是4点或者以上,要不然他将选择重掷3颗骰子.根据以上分析,满足条件的情况有:(1)掷出1点、6点、6点,3种情况;(2)掷出2点、5点、5点,3种情况;(3)掷出2点、5点、6点,6种情况;(4)掷出2点、6点、6点,3种情况;(5)掷出3点、4点、4点,3种情况;(6)掷出3点、4点、5点,6种情况;(7)掷出3点、4点、6点,6种情况;(8)掷出3点、5点、5点,3种情况;(9)掷出3点、5点、6点,6种情况;(10)掷出3点、6点、6点,3种情况.由加法原理,共42种情况,故所求概率为42216=736,(A)正确.。
2021年美国数学竞赛(AMC10A)的试题与解答

2021年美国数学竞赛(AMC10A)的试题与解答华南师范大学数学科学学院(510631)李湖南1.算式(22−2)−(32−3)+(42−4)的值是多少?(A)1(B)2(C)5(D)8(E)12解直接计算,原式=2−6+12=8,故(D)正确.2.Portia高中的学生人数是Lara高中的3倍,这两所高中总共有2600名学生.问Portia高中有多少名学生?(A)600(B)650(C)1950(D)2000(E)2050解依题意可知,Portia高中占总人数的34,即有2600×34=1950名学生,故(C)正确.3.两个自然数之和是17402.这两个数中的一个可以被10整除,如果去掉该数的个位数字则得到另外一个数.问这两个数的差是多少?(A)10272(B)11700(C)13362(D)14238(E)15426解设第一个数为x,则第二个数为x10,已知x+x10=17402,解得x=15820,于是两数之差为x−x10=910x=14238,故(D)正确.4.一辆小车冲下山坡,它第一秒移动了5英寸,并且速度不断加快.在每个连续的1秒时间间隔内,它都比前1秒多移动7英寸.小车用了30秒到达山脚.问它一共行进了多少英寸?(A)215(B)360(C)2992(D)3195(E)3242解小车每秒移动的距离是个等差数列,首项为5,公差为7,第30秒移动了208英寸,从而距离和为(5+208)×30÷2=3195英寸,故(D)正确.5.一个共有k>12名学生的班级进行测验的平均分为8分,其中12名学生的测验平均分是14分.问其余学生的测验平均分如何用k来表示?(A)14−8k−12(B)8k−168k−12(C)1412−8k(D)14(k−12)k2(E)14(k−12)8k解所有学生的分数总和为8k,其中12名学生的分数和为168,剩下k−12名学生的分数和为8k−168,故平均分为8k−168k−12,(B)正确.6.Chantal和Jean从山路的起点开始向消防塔徒步旅行.Jean背着一个沉重的背包,走得较慢.Chantal开始以每小时4英里的速度行走,走到路程一半,山路变得非常陡峭,Chantal的速度减慢到每小时2英里.到达塔后,她立即掉头,以每小时3英里的速度沿着陡峭的山路向下走,她在路程的一半处遇见了Jean.从出发到他们相遇,Jean的平均速度是每小时多少英里?(A)1213(B)1(C)1312(D)2413(E)2解设半程为x英里,Jean所用的时间与Chantal一样,均为x4+x2+x3=13x12小时,于是Jean的平均速度为x(13x)/12=1213英里/小时,故(A)正确.7.汤姆有13条蛇,其中4条是紫色的,5条是快乐的.他观察发现:他的所有快乐的蛇都能做加法,他的紫色的蛇不会做减法,而且他所有不会做减法的蛇也不会做加法.关于汤姆的蛇,可以得出以下哪个结论?(A)紫色的蛇可以做加法(B)紫色的蛇是快乐的(C)能做加法的蛇是紫色的(D)快乐的蛇不是紫色的(E)快乐的蛇不能做减法解紫色的蛇不会做减法,从而也不会做加法,因此也不是快乐的.故快乐的蛇都不是紫色的,(D)正确.8.一名学生在用66乘以如下的循环小数时, 1.abab···=1.˙a˙b,其中a和b是数字.他没有注意到循环小数的标识,而只是做了66乘以1.ab.后来他发现他的答案比正确答案小0.5.问两位整数ab是多少?(A)15(B)30(C)45(D)60(E)75解由题意可得,0.5=66×(1.˙a˙b−1.ab)=66×0.00˙a˙b= 0.66×ab99,解得ab=75,故(E)正确.9.对于实数x和y,(xy−1)2+(x+y)2的最小可能值是多少?(A)0(B)14(C)12(D)1(E)2解(xy−1)2+(x+y)2=x2y2+x2+y2+1 1,此时x=y=0,故(D)正确.10.算式(2+3)(22+32)(24+34)(28+38)(216+ 316)(232+332)(264+364)与下面哪个表达式相等?(A)3127+2127(B)3127+2127+2×363+3×263(C)3128−2128(D)3128+2128(E)5127解连续使用平方差公式,可得原式=(3−2)(3+2)(32+22)(34+24)(38+28)(316+216)(332+232)(364+264)=3128−2128,故(C)正确.11.选择下面哪个整数b 为基数,可以使得b 进制数2021b −221b 不能被3整除?(A)3(B)4(C)6(D)7(E)8解由于2021b −221b =2×b 3−2×b 2=2b 2(b −1),当b =3,4,6,7时,该数均能被3整除,故(E)正确.12.如图所示,两个顶点朝下的正圆锥包含相同量的液体.液体顶部表面的半径分别为3厘米和6厘米.在每个圆锥体中放入一个半径为1厘米的球形弹子,它沉入底部,完全浸没,没有任何液体溢出.问窄圆锥内液面上升的高度与宽圆锥内液面上升的高度之比是多少?(A)1:1(B)47:43(C)2:1(D)40:13(E)4:1解设液体的体积为V ,左右两边液面高度分别为h 1,h 2,则V =13π32·h 1=13π62·h 2,因而h 1=4h 2.设放入弹子的体积为V 0=43π,左右两边液面上升高度分别为∆h 1,∆h 2,顶部液面的半径分别为r 1,r 2,则有V +V 0=13πr 21·(h 1+∆h 1)=13πr 22·(h 2+∆h 2),3r 1=h 1h 1+∆h 1,6r 2=h 2h 2+∆h 2,解得r 1r 2=12,∆h 1∆h 2=41,故(E)正确.13.四面体ABCD 中,各边长为AB =2,AC =3,AD =4,BC =√13,BD =2√5和CD =5,问它的体积是多少?(A)3(B)2√3(C)4(D)3√3(E)6解如图所示,由于AB 2+AC 2=BC 2,AB 2+AD 2=BD 2,AC 2+AD 2=CD 2,可得AB,AC,AD 互相垂直,即ABCD 是个直四面体.故V ABCD =13S ∆ABD ·AC =13·12×2×4·3=4,(C)正确.14.多项式z 6−10z 5+Az 4+Bz 3+Cz 2+Dz +16的根都是正整数,有可能重复.问B 的取值是多少?(A)−88(B)−80(C)−64(D)−41(E)−40解设多项式的根为x 1,x 2,x 3,x 4,x 5,x 6,其中均为正整数,则z 6−10z 5+Az 4+Bz 3+Cz 2+Dz +16=(z −x 1)(z −x 2)···(z −x 6),可得 x 1+x 2+x 3+x 4+x 5+x 6=10,x 1x 2x 3x 4x 5x 6=16,−∑i<j<kx i x j x k =B,解得x 1=x 2=1,x 3=x 4=x 5=x 6=2,于是B =−(C 14·12·2+C 12·C 24·1·22+C 34·23)=−88,故(A)正确.15.A,B,C 和D 的值从{1,2,3,4,5,6}中不重复地选取(即没有两个字母的取值相同),使得两条曲线y =Ax 2+B 和y =Cx 2+D 相交的不同取值方式有多少种?(不考虑曲线列出的顺序,例如,A =3,B =2,C =4,D =1与A =4,B =1,C =3,D =2被认为是相同的)(A)30(B)60(C)90(D)180(E)360解要使得y =Ax 2+B 和y =Cx 2+D 相交,则方程Ax 2+B =Cx 2+D 有解,即有(A −C )x 2=D −B ,此时A −C,D −B 的符号一样.任取A,C ∈{1,2,3,4,5,6},则D,B ∈{1,2,3,4,5,6}−{A,C },大小关系须一致,则有2C 26C 24=180种选择;另外不考虑曲线的顺序,故不同的取值方式共有180÷2=90种,(C)正确.16.在下面的数据列表中,对于1 n 200,整数n 出现了n 次.1,2,2,3,3,3,4,4,4,4,···,200,200,···,200.问这组数据列表中的中位数是多少?(A)100.5(B)134(C)142(D)150.5(E)167解这列数共有1+2+3+ (200)12×200×201=20100个,中位数是第10050与第10051个数的平均值.先求得12n (n +1) 10050的最大值为n max =141,且12n max (n max +1)=10011.这说明第10050和第10051个数均为142,故中位数为142,(C)正确.17.在梯形ABCD 中,AB //CD ,BC =CD =43,并且AD ⊥BD .设O 是对角线AC 和BD 的交点,P 是BD的中点.已知OP =11,AD 的长度可以表示成m √n ,其中m 和n 是正整数,并且n 不能被任何质数的平方所整除.问m +n 的值是多少?(A)65(B)132(C)157(D)194(E)215解如图所示,延长CP 交AB 于点E ,由于∆CBD 是等腰三角形,P 是BD 中点,从而CP⊥BD ,进而CE //DA ,于是AECD 是个平行四边形,得EA =CD =43.又∠CBD =∠CDB =∠ABD ,可得∆CBP =∆EBP ,即得BE =BC =43,于是AB =86.再根据∆CDO ∆ABO ,有OD OB =CD AB =12,即OD OB =BP −11BP +11=12,解得BP =33,从而AD =CE =2CP =2√BC 2−BP 2=2√432−332=4√190,故m +n =194,(D)正确.18.令f 是一个定义在正有理数集合上的函数,它具有性质:对于所有的正有理数a 和b ,f (ab )=f (a )+f (b ).假设f 还具有性质:对于每一个质数p ,f (p )=p .问以下哪个数x 满足f (x )<0?(A)1732(B)1116(C)79(D)76(E)2511解f (1)=f (1·1)=2f (1)⇒f (1)=0,f (1)=f (b ·1b )=f (b )+f (1b )=0⇒f (1b)=−f (b ),对于任意正有理数b ;f (a b )=f (a ·1b )=f (a )+f (1b)=f (a )−f (b ),对于任意正有理数a 和b ;f (p n )=f (p ·p ·····p n 个)=nf (p ),对于任意质数p ,n ∈N .因此,f (2511)=f (25)−f (11)=2f (5)−f (11)=2·5−11=−1<0,故(E)正确.19.由x 2+y 2=3|x −y |+3|x +y |的图像所界定的图形的面积是m +nπ,其中m 和n 是整数.问m +n 是多少?(A)18(B)27(C)39(D)45(E)54解如图所示,对点(x,y )的位置进行讨论:(1)在第一象限:当y x 时,方程为x 2+y 2=3(x −y )+3(x +y ),化简可得(x −3)2+y 2=9,它是一个八分之一圆;当y >x 时,方程可化为x 2+(y −3)2=9,也是一个八分之一圆;(2)在第二象限:当y −x 时,方程可化为x 2+(y −3)2=9;当y <−x 时,方程可化为(x +3)2+y 2=9;(3)在第三象限:当y x 时,方程可化为(x +3)2+y 2=9;当y <x 时,方程可化为x 2+(y +3)2=9;(4)在第四象限:当y −x 时,方程可化为(x −3)2+y 2=9;当y <−x 时,方程可化为x 2+(y +3)2=9.综上可得,该图像由四个半径为3的半圆封闭而成,面积为62+2·π·32=36+18π,故m +n =36+18=54,(E)正确.20.数列1,2,3,4,5有多少种重新排列的方式,使得没有连续三项是递增的,也没有连续三项是递减的?(A)10(B)18(C)24(D)32(E)44解由题意可知,符合条件的数列中,连续两项的单调性只能是:增减增减,或减增减增.用排列表示即有:13254,14253,14352,15243,15342;21435,21534,23154,24153,24351,25143,25341;31425,31524,32415,32514,34152,34251,35142,35241.如果对集合{1,2,3,4,5}做一个置换1234554321 ,所得排列仍然符合条件,即以5,4开头的排列和以1,2开头的排列一样多.故所有符合条件的数列有(5+7)×2+8=32个,(D)正确.21.设ABCDEF 是等角六边形,由直线AB ,CD 和EF 所组成的三角形面积为192√3,由直线BC ,DE 和F A 所组成的三角形的面积是324√3.六边形ABCDEF 的周长可用m +n √p 表达,其中m ,n 和p 是正整数,并且p不能被任何质数的平方整除.问m +n +p 的值是多少?(A)47(B)52(C)55(D)58(E)63解如图所示,分别向两边延长各边,交于点G ,H ,I ,J ,K ,L ,由题意可得,S ∆GHI =192√3,S ∆JKL =324√3.由于六边形的每个内角都是120◦,因此图中所有三角形的内角都是60◦,即所有三角形都是等边三角形.于是,S ∆GHI =√34IG 2=√34(a +b +f )2=192√3,解得a +b +f =16√3;同理S ∆JKL =√34(c +d +e )2=324√3,得c +d +e =36.故六边形的周长为a +b +c +d +e +f =36+16√3,即m +n +p =36+16+3=55,(C)正确.22.Hiram 的代数笔记有50页,打印在25张纸上;第一张纸包括第1和第2页,第二张纸包括第3和第4页,以此类推.有一天,他去午餐前把笔记本放在桌子上,室友决定从笔记中间借几页.当Hiram回来时,他发现他的室友从笔记中拿走了连续的若干张纸,并且所有剩余纸张上页码的平均值正好是19.问有多少张纸被借走了?(A)10(B)13(C)15(D)17(E)20解设笔记被借走了x张纸,分别是从第a张纸到第a+x−1张纸,其中a+x−1 25,页码正好是从2a−1到2(a+x−1),页码和为(2a−1)+2a+···+2(a+x−1)=(2a−1)+2(a+x−1)2·2x=(4a+2x−3)x,而所有页码和为1+2+···+50=1275,从而剩下的页码和为19(50−2x)=1275−(4a+2x−3)x,整理得(4a+2x−41)x=325,解得x=13,a=10,故(B)正确.23.青蛙Frieda在一个3×3的方格表上开始一系列跳跃,每次跳跃都随机选择一个方向—–向上、向下、向左或向右,从一个方格移动到旁边的方格.她不能斜着跳,当跳跃的方向会使得Frieda离开方格表时,她会“绕个圈”,跳到相对的另一边.例如,如果Frieda从中心方格开始,向上跳跃两次,第一次跳跃后她将位于最上面一行的中间方格,第二次跳跃将使得Frieda跳到相对的边,落在最下面一行的中间方格.假设Frieda从中心方格出发,最多随机跳跃四次,并且当到达角落方格时就停止跳跃.问她在四次跳跃中到达角落方格的概率是多少?(A)916(B)58(C)37(D)2532(E)1316解记P(n)为第n次到达角落方格的概率,显然P(1)=0;第一次跳跃可朝四个方向,不妨设第一次跳跃向右,则当第二次跳跃向上或向下时,可到达角落方格,概率为24,即P(2)=12;若第二次跳跃向左,概率为14,即回到出发点S,此时第四次跳跃到达角落方格的概率为1 4×P(2)=18;若第二次跳跃向右,概率为14,此时到了S左边的方格,则第三次跳跃向上或向下可到达角落方格,即P(3)=14×12=18;若第三次跳跃向左,概率为14,则回到S右边的方格,第四次跳跃向上或向下可到达角落方格,概率为14×14×12=132,从而P(4)=18+132=532.而其它情况均四次到达不了角落方格.故所求概率为4∑i=1P(i)=0+12+18+532=2532,(D)正确.24.设a是正实数,考虑由(x+ay)2=4a2和(ax−y)2=a2组成的四边形的内部.对所有的a>0而言,这个区域的面积怎样用a来表示?(A)8a2(a+1)2(B)4aa+1(C)8aa+1(D)8a2a2+1(E)8aa2+1解如图所示,四边形由四条直线y=−1ax±2,y=ax±a围成,由于斜率之积为−1,从而四边形是个矩形.设直线y=−1ax+2与x轴负方向的夹角为θ,则tanθ=1a,矩形的长为4cosθ,宽为2cosθ,即所求面积为S=8cos2θ.于是S=4·2cos2θ=4(1+cos2θ)=4(1+1−tan2θ1+tan2θ)=4·21+tan2θ=8·11+1a2=8a21+a2.故(D)正确.25.将3枚不可区分的红色筹码,3枚不可区分的蓝色筹码和3枚不可区分的绿色筹码分别放入3×3方格表的各个小方格中,使得无论是垂直方向还是水平方向,都没有两个相同颜色的筹码相邻,问共有多少种放法?(A)12(B)18(C)24(D)30(E)36解首先考虑中心方格,不妨设放入红色筹码,分两种情况:(1)其余两个红色筹码在一边,四个方向均可:那剩下6个方格只能按以下方式放入,∆放一种颜色,空格放另一种颜色.此时有4×2=8种放法;(2)其余两个红色筹码各在一边,即在对角线上,两个方向均可:那剩下6个方格也只能按以下方式放入,∆放一种颜色,空格放另一种颜色.此时有2×2=4种放法.综上,中心方格放入红色筹码,共有12种放法.如果放入蓝色筹码或绿色筹码,也是一样,故所有的放法有3×12=36种,(E)正确.。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
2011AMC10美国数学竞赛A卷时间:2021.03.03 创作:欧阳学1. A cell phone plan costs $20 each month, plus 5¢per text message sent, plus 10¢ for each minute used over 30 hours. In January Michelle sent 100 text messages and talked for 30.5 hours. How much did she have to pay?(A) $24.00(B) $24.50(C) $25.50(D) $28.00(E) $30.002. A small bottle of shampoo can hold 35 milliliters of shampoo, Whereas a large bottle can hold 500 milliliters of shampoo. Jasmine wants to buy the minimum number of small bottles necessary to completely fill a large bottle. How many bottles must she buy?(A) 11(B) 12(C) 13(D) 14(E) 153. Suppose [a b] denotes the average of a and b, and {a b c} denotes the average of a, b, and c. What is {{1 1 0} [0 1] 0}?(A)(B)(C)(D)(E)4. Let X and Y be the following sums of arithmetic sequences: X= 10 + 12 + 14 + …+ 100.Y= 12 + 14 + 16 + …+ 102.What is the value of ?(A) 92(B) 98(C) 100(D) 102(E) 1125. At an elementary school, the students in third grade, fourth grade, and fifth grade run an average of 12, 15, and 10 minutes per day, respectively. There are twice as many third graders as fourth graders, and twice as many fourth graders as fifth graders. What is the average number of minutes run per day by these students?(A) 12(B) (C) (D) 13 (E) 146. Set A has 20 elements, and set B has 15 elements. What is the smallest possible number of elements in A∪B, the union of A and B?(A) 5(B) 15(C) 20(D) 35 (E) 3007. Which of the following equations does NOT have a solution?(A) (B) (C)(D) (E)8. Last summer 30% of the birds living on TownLake were geese, 25% were swans, 10% were herons, and 35% were ducks. What percent of the birds that were not swans were geese?(A) 20(B) 30(C) 40(D) 50(E) 609. A rectangular region is bounded by the graphs of the equations y=a, y=-b, x=-c, and x=d, where a, b, c, and d are allpositive numbers. Which of the following represents the area of this region?(A) ac + ad + bc + bd(B) ac – ad + bc – bd(C) ac + ad – bc –bd(D) –ac –ad + bc + bd(E) ac – ad – bc + bd10. A majority of the 20 students in Ms. Deameanor’s class bought pencils at the school bookstore. Each of these students bought the same number of pencils, and this number was greater than 1. The cost of a pencil in cents was greater than the number of pencils each student bought, and the total cost of all the pencils was $17.71. What was the cost of a pencil in cents?(A) 7(B) 11(C) 17(D) 23 (E) 7711. Square EFGH has one vertex on each side of square ABCD. Point E is on AB with AE=7·EB. What is the ratio of the area of EFGH to the area of ABCD?(A)(B)(C)(D)(E)12. The players on a basketball team made some three-point shots, some two-point shots, some one-point free throws. They scored as many points with two-point shots as with three-point shots. Their number of successful free throws was one morethan their number of successful two-point shots. The team’s total score was 61 points. How many free throws did they make?(A) 13(B) 14(C) 15(D) 16(E) 1713. How many even integers are there between 200 and 700 whose digits are all different and come from the set {1, 2, 5, 7, 8, 9}?(A) 12(B)20(C)72(D) 120 (E) 20014. A pair of standard 6-sided fair dice is rolled once. The sum of the numbers rolled determines the diameter of a circle. What is the probability that the numerical value of the area of the circle is less than th e numerical value of the circle’s circumference?(A)(B)(C)(D)(E)15. Roy bought a new battery-gasoline hybrid car. On a trip the car ran exclusively on its battery for the first 40 miles, then ran exclusively on gasoline for the rest of the trip, using gasoline at a rate of 0.02 gallons per mile. On the whole trip he averaged 55 miles per gallon. How long was the trip in miles?(A) 140(B) 240(C) 440(D) 640(E) 84016. Which of the following in equal to ?(A)(B)(C)(D)(E)17. In the eight-term sequence A, B, C, D, E, F, G, H, the value of C is 5 and the sum of any three consecutive terms is 30. What is A + H?(A) 17(B) 18(C) 25(D) 26 (E) 4318. Circles A, B, and C each have radius 1. Circles A and B share one point of tangency. Circle C has a point of tangency with the midpoint of AB. What is the area inside Circle C but outside Circle A and Circle B?(A)(B)(C)(D)(E)19. In 1991 the population of a town was a perfect square. Ten years later, after an increase of 150 people, the population was 9 more than a perfect square. Now, in 2011, with an increase of another 150 people, the population is once again a perfect square. Which of the following is closest to the percent growth of the town’s population during this twenty-year period?(A) 42(B) 47(C) 52(D) 57 (E) 6220. Two points on the circumference of a circle of radius r are selected independently and at random. From each point a chord of length r is drawn in a clockwise direction. What is the probability that the two chords intersect?(A)(B)(C)(D)(E)21. Two counterfeit coins of equal weight are mixed with 8 identical genuine coins. The weight of each of the counterfeit coins is different from the weight of each of the genuine coins.A pair of coins is selected at random without replacement from the 10 coins. A second pair is selected at random without replacement from the remaining 8 coins. The combined weight of the first pair is equal to the combined weight of the second pair. What is the probability that all 4 selected coins are genuine?(A)(B)(C)(D)(E)22. Each vertex of convex pentagon ABCDE is to be assigneda color. There are 6 colors to choose from, and the ends of each diagonal must have different colors. How many different colorings are possible?(A) 2500(B) 2880(C) 3120(D) 3250 (E) 375023. Seven students count from 1 to 1000 as follows:·Alice says all the numbers, except she skips the middle number in each consecutive group of three numbers. That is Alice says 1, 3, 4, 6, 7, 9, …, 997, 999, 1000.·Barbara says all of the numbers that Alice doesn’t say, except she also skips the middle number in each consecutivegrope of three numbers.·Candice says all of the numbers that neither Alice nor Barbara says, except she also skips the middle number in each consecutive group of three numbers.·Debbie, Eliza, and Fatima say all of the numbers that none of the students with the first names beginning before theirs in the alphabet say, except each also skips the middle number in each of her consecutive groups of three numbers.·Finally, George says the only number that no one else says. What number does George say?(A) 37(B) 242(C) 365(D) 728 (E) 99824. Two distinct regular tetrahedra have all their vertices among the vertices of the same unit cube. What is the volume of the region formed by the intersection of the tetrahedra?(A)(B)(C)(D)(E)25. Let R be a square region and an integer. A point X in the interior of R is called n-ray partitional if there are n rays emanating from X that divide R into N triangles of equal area. How many points are 100-ray partitional but not 60-ray partitional?(A) 1500(B) 1560(C) 2320(D) 2480 (E) 25002011AMC10美国数学竞赛A卷1. 某通讯公司手机每个月基本费为20美元, 每传送一则简讯收 5美分(一美元=100 美分)。
