Igcse-数学-历年真题

合集下载

IGCSE真题_20140116

Turn over P42864A©2014 Pearson Education Ltd.1/1/1/1/*P42864A0120*Instructionst Use black ink or ball-point pen.t Fill in the boxes at the top of this page with your name,centre number and candidate number.t Answer all questions.t A nswer the questions in the spaces provided– there may be more space than you need.t Show all the steps in any calculations and state the units.t SInformationt The total mark for this paper is 60.t T he marks for each question are shown in brackets– use this as a guide as to how much time to spend on each question.Advicet Read each question carefully before you start to answer it.t Keep an eye on the time.t Write your answers neatly and in good English.t Try to answer every question.t Check your answers if you have time at the end.2*P42864A0220*3*P42864A0320*Turn overBLANK PAGE4*P42864A0420*5*P42864A0520*Turn over6*P42864A0620*2 Bromine, chlorine, fluorine and iodine are elements in Group 7 of the Periodic Table.(a) Which two of these elements have the darkest colours?(1)....................................................................................................................................and ....................................................................................................................................(b) The equation for the reaction between hydrogen and chlorine isH 2 + Cl 2 o 2HClDifferent names are used for the product, depending on its state symbol.(i) What are the names used for HCl(g) and HCl(aq)?(2)HCl(g) .................................................................................................................................................................................................................................................................HCl(aq) ............................................................................................................................................................................................................................................................... (ii) The presence of HCl(g) can be confirmed by adding ammonia (NH 3) gas.State the observation in the reaction between HCl(g) and ammonia gas and write a chemical equation for the reaction.(2)observation ......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................chemical equation ................................................................................................................................................................................................................................ (iii) The presence of chloride ions in HCl(aq) can be shown by mixing it with silvernitrate solution and dilute nitric acid.State the result of this test and complete the chemical equation for the reaction by adding the state symbols.(3)result .......................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................AgNO 3(.......................) + HCl(aq) o AgCl(.......................) + HNO 3(.......................)7*P42864A0720*Turn over8*P42864A0820*3 Tungsten is a useful metal. It has the chemical symbol W. (a) One method of extracting tungsten involves heating a tungsten compound (WO 3)with hydrogen.(i) Suggest the chemical name of WO 3(1)....................................................................................................................................................................................................................................................................................(ii) Balance the equation for the reaction between WO 3 and hydrogen.(1)WO 3 + ............................H 2 o ............................W + ............................H 2O(iii) Why is this reaction described as reduction?(1)........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(b) Scheelite is an ore of tungsten. The main compound in scheelite has the percentage composition by mass Ca = 13.9%, W = 63.9%, O = 22.2%.Calculate the empirical formula of this compound.(3)empirical formula = ...................................................9*P42864A0920*Turn over(c) Tungsten can also be obtained by reacting tungsten fluoride with hydrogen.The equation for this reaction isWF 6 + 3H 2 o W + 6HF(i) In an experiment, a chemist used 59.6g of tungsten fluoride. What is the maximum mass of tungsten he could obtain from 59.6 g of tungsten fluoride?Relative formula mass of tungsten fluoride = 298(2)maximum mass = ................................................... g(ii) Starting with a different mass of tungsten fluoride, he calculates that the massof tungsten formed should be 52.0 g. In his experiment he actually obtains 47.5 g of tungsten.What is the percentage yield of tungsten in this experiment?(2)percentage yield = ................................................... %(Total for Question 3 = 10 marks)10*P42864A01020*4 A student investigated the neutralisation of acids by measuring the temperature changeswhen alkalis were added to acids of known concentrations.He used this apparatus to add different volumes of sodium hydroxide solution to a fixed volume of dilute nitric acid.He used this method. Ɣ measure the temperature of 25.0 cm 3 of the acid in the polystyrene cupƔ add the sodium hydroxide solution in 5.0 cm 3 portions until a total of 30.0 cm 3has been added(a) State two properties of the sodium hydroxide solution that should be kept constantfor each 5.0 cm 3 portion.(2)1 ...................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................2 ...................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................polystyrene cup11*P42864A01120*Turn over12*P42864A01220*13*P42864A01320*Turn over14*P42864A01420*(f) Another student used sulfuric acid instead of nitric acid in her experiments.She started with 25.0 cm 3 of sulfuric acid of concentration 0.650 mol/dm 3. She added 0.500 mol/dm 3 sodium hydroxide solution until the acid was completely neutralised.The equation for this reaction is2NaOH + H 2SO 4 o Na 2SO 4 + 2H 2O(i) Calculate the amount, in moles, of sulfuric acid used.(2)amount = ................................. mol(ii) Calculate the amount, in moles, of sodium hydroxide needed to neutralise thisamount of sulfuric acid.(1)amount = ................................. mol(iii) Calculate the volume, in cm 3, of sodium hydroxide solution needed to neutralisethis amount of sulfuric acid.(2)volume = ................................. cm 3(Total for Question 4 = 18 marks)15*P42864A01520*Turn over16*P42864A01620*(c) The equation for one reaction that could occur in process 2 is C x H y o C 5H 12 + 2C 2H 4(i) Deduce the formula of C x H y(1)....................................................................................................................................................................................................................................................................................(ii) Give the name of the compound C 5H 12(1)....................................................................................................................................................................................................................................................................................(iii) Draw the displayed formula of C 2H 4(1)(d) The structural formula of chloroethene formed in process 3 is CH 2CHClThe polymer formed in process 4 is poly(chloroethene).Draw the displayed formula for the repeat unit of poly(chloroethene).(2)17*P42864A01720*(e) Poly(chloroethene) is formed by addition polymerisation. Nylon is formed by condensation polymerisation.(i) How does condensation polymerisation differ from addition polymerisation?(1)........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(ii) Poly(chloroethene) and nylon do not biodegrade easily.What is meant by the term biodegrade ?(2)................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(iii) What feature of addition polymers makes it difficult for them to biodegrade?(1)........................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................................(Total for Question 5 = 13 marks)(TOTAL FOR PAPER = 60 MARKS)18*P42864A01820*BLANK PAGE19*P42864A01920*BLANK PAGE20*P42864A02020*BLANK PAGE。

2024 GRE考试必备数学历年真题练习

2024 GRE考试必备数学历年真题练习在GRE数学部分的备考过程中，历年真题的练习是非常重要的一环。

通过针对性的练习，考生可以熟悉考试题型，了解考点，提升解题速度和准确性。

本文将为大家提供2024年GRE考试的数学历年真题练习，帮助考生更好地备考。

1. 整数1.1 题目选择下列哪个数是正偶数？(A) -12(B) -5(C) 0(D) 9(E) 271.2 解析正偶数是指能够被2整除的正整数。

从选项中排除负数，在0和正数中，只有0能够被2整除，因此答案选(C)。

2. 几何2.1 题目下图中，正方形ABCD的边长为3。

点E是线段BC的中点，点F是线段BD上的一点，且AF的长度为3。

求射线AF与线段CE的交点P到点E的距离。

[图片描述：一个正方形ABCD，边长为3，线段BC的中点为E，线段BD上的一点为F，AF的长度为3]2.2 解析首先，可以得出正方形ABCD的对角线AC的长度为3的开平方乘以2，即AC=3乘以根号2。

由于AE与CF平行且等长，射线AF可以看作与线段BE平行且等长。

因此，三角形BEP是等腰直角三角形，所以BP = EP = EC的一半。

又因为BC=3，所以EC=3/2。

因此，点P到点E的距离为1.5个单位。

3. 概率与统计3.1 题目某次测试的成绩服从正态分布，平均成绩为80分，标准差为5分。

已知一个学生的成绩在85分以上的概率为0.841，求这个学生的成绩。

3.2 解析根据正态分布的性质，均值加上标准差得到的分数对应的概率是大约0.841。

因此，这个学生的成绩应该在平均成绩80分加上标准差5分的位置，即85分。

通过以上三个部分的例题，希望能够帮助到考生更好地了解2024年GRE考试数学部分的题型和解题思路。

在备考过程中，考生还需深入学习数学知识，掌握解题技巧，并进行大量真题练习，提升解题能力。

祝愿各位考生在考试中取得好成绩！。

Igcse-数学-历年真题-2

4400/4HEdexcel IGCSEMathematicsPaper 4HHigher TierFriday 11 June 2010 – AfternoonTime: 2 hoursMaterials required for examination Items included with question papersRuler graduated in centimetres and Nilmillimetres, protractor, compasses,pen, HB pencil, eraser, calculator.Tracing paper may be used.Instructions to CandidatesIn the boxes above, write your centre number, candidate number, your surname, initials and signature.Check that you have the correct question paper.Answer ALL the questions. Write your answers in the spaces provided in this question paper.You must NOT write on the formulae page. Anything you write on the formulae page will gain NO credit.If you need more space to complete your answer to any question, use additional answer sheets.Information for CandidatesThe marks for individual questions and the parts of questions are shown in round brackets: e.g. (2).There are 22 questions in this question paper. The total mark for this paperis 100.You may use a calculator.Advice to CandidatesWrite your answers neatly and in good English.This publication may be reproduced only in accordance with Edexcel Limited copyright policy.©2010 Edexcel Limited.Printer’s Log. No. N36905AIGCSE MATHEMATICS 4400 FORMULA SHEET – HIGHER TIERAnswer ALL TWENTY TWO questions.Write your answers in the spaces provided.You must write down all stages in your working.1. Solve 6 y – 9 = 3 y + 7y = ................................(Total 3 marks) 2. The diagram shows two towns, A and B, on a map.(a) By measurement, find the bearing of B from A.....................................︒(2)C is another town.The bearing of C from A is 050︒.(b) Find the bearing of A from C.....................................︒(2) (Total 4 marks)3. A spinner can land on red or blue or yellow.The spinner is biased.The probability that it will land on red is 0.5The probability that it will land on blue is 0.2Imad spins the spinner once.(a) Work out the probability that it will land on yellow......................................(2)Janet spins the spinner 30 times.(b)Work out an estimate for the number of times the spinner will land on blue......................................(2)(Total 4 marks)4. Rosetta drives 85 kilometres in 1 hour 15 minutes.(a) Work out her average speed in kilometres per hour...................................... km/h(2)Rosetta drives a total distance of 136 kilometres.(b) Work out 85 as a percentage of 136................................. %(2)Sometimes Rosetta travels by train to save money.The cost of her journey by car is £12The cost of her journey by train is 15% less than the cost of her journey by car.(c)Work out the cost of Rose tta’s journey by train.£ ...................................(3)(Total 7 marks)5.Calculate the value of x.Give your answer correct to 3 significant figures.x = ................................(Total 3 marks)6. A = {2, 3, 4, 5}B = {4, 5, 6, 7}(a)(i) List the members of A ⋂B......................................(ii) How many members are in A ⋃B?.....................................(2)ℰ = {3, 4, 5, 6, 7}P = {3, 4, 5}Two other sets, Q and R, each contain exactly three members.P ⋂Q = {3, 4}P ⋂R = {3, 4}Set Q is not the same as set R.(b)(i) Write down the members of a possible set Q......................................(ii) Write down the members of a possible set R......................................(2)(Total 4 marks)7. Rectangular tiles have width (x + 1) cm and height (5x – 2) cm.Some of these tiles are used to form a large rectangle.The large rectangle is 7 tiles wide and 3 tiles high.The perimeter of the large rectangle is 68 cm.(a) Write down an equation in x...............................................................................................................(3)(b) Solve this equation to find the value of x.x = ................................(3)(Total 6 marks)8. Show that 121 141 = 1519. The depth of water in a reservoir increases from 14 m to 15.75 m.Work out the percentage increase.................................. %(Total 3 marks) 10. Quadrilaterals ABCD and PQRS are similar.AB corresponds to PQ.BC corresponds to QR.CD corresponds to RS.Find the value of(a) xx = ...............................(2)(b) yy = ...............................(1)(Total 3 marks)11. Simplify fully6x + 43x.....................................(Total 3 marks)12.(a)Find the equation of the line L......................................(3)(b) Find the three inequalites that define the unshaded region shown in the diagram below................................................................................................................(3)(Total 6 marks)13. (a) Solve x 2– 8x + 12 = 0.....................................(3)(b) Solve the simultaneous equationsy = 2x4x – 5y = 9x = ................................y = ................................(3)(Total 6 marks)14.The area of the triangle is 6.75 cm2.The angle x° is acute.Find the value of x.Give your answer correct to 1 decimal place.x = ................................(Total 3 marks)15. The unfinished histogram shows information about the heights, h metres, ofsome trees.(a) Calculate an estimate for the number of trees with heights in theinterval 4.5 < h ≤ 10.....................................(3)(b) There are 75 trees with heights in the interval 10 < h ≤ 13Use this information to complete the histogram.(2)(Total 5 marks)16. A bag contains 3 white discs and 1 black disc.John takes at random 2 discs from the bag without replacement.(a) Complete the probability tree diagram.First disc Second disc(3)(b)Find the probability that both discs are white......................................(2)All the discs are now replaced in the bag.Pradeep takes at random 3 discs from the bag without replacement.(c)Find the probability that the disc left in the bag is white......................................(3)(Total 8 marks)17. The diagram s hows a sector of a circle, radius 45 cm, with angle 84°.Calculate the area of the sector.Give your answer correct to 3 significant figures.............................. cm2(Total 3 marks) 18.Calculate the length of AC.Give your answer correct to 3 significant figures................................ cm(Total 3 marks)19. A cone has slant height 4 cm and base radius r cm.The total surface area of the cone is 433π cm 2.Calculate the value of r .r = ................................(Total 4 marks)20. f(x) = (x – 1)2(a) Find f(8).....................................(1)The domain of f is all values of x where x ≥ 7(a)Find the range of f......................................(2)xg(x) =x1(c) Solve the equation g(x) = 1.2.....................................(2)(d) (i) Express the inverse function g –1 in the form g –1(x) = .......g –1(x) = ...................................(ii) Hence write down gg(x) in terms of x.gg(x) = ....................................(6)(Total 11 marks)21.In the diagram = a and = c.(a) Find CA in terms of a and c......................................(1)The point B is such that AB=1c.2(b) Give the mathematical name for the quadrilateral OABC......................................(1)The point P is such that = a + k c, where k ≥ 0(c) State the two conditions relating to a + k c that must be true for OAPCto be a rhombus.(2)(Total 4 marks)22. (a) Work out 5.2 × 102+ 2.3 × 104Give your answer in standard form......................................(2)a × 102 +b × 104 =c × 104(b) Express c in terms of a and b.c = ................................(2)(Total 4 marks)TOTAL FOR PAPER = 100 MARKS END。

igcse数学练习题

igcse数学练习题在本文中，将为您提供一系列IGCSE数学练习题，以帮助您巩固和提高数学知识和技能。

这些练习题的题型多样，涵盖了IGCSE数学考试的各个知识点和难度级别。

一、选择题1. 在下列数中，哪个数是一个自然数？A. -3B. 2/3C. 0D. √22. 将下列分数化为最简形式：8/12A. 1/2B. 4/6C. 2/3D. 3/43. 当x = 2时，下列哪个等式成立？A. 5x - 3 = 7B. 3x + 2 = 10C. 4x + 1 = 8D. 2x + 5 = 124. 一个三角形的面积是36平方单位，底边长为6单位，高边长为x单位。

求x的值。

A. 3B. 9C. 12D. 18二、填空题1. 用两个相邻整数的平方的和表示一个奇数的方式是：_________。

2. 一个长方形的长为x+2，宽为x-3，其面积可以表示为_________。

三、解答题1. 求解方程：2x + 5 = 17。

2. 计算:(a + b)^2，其中a = 3，b = -2。

3. 解决以下三角形相似问题：两个三角形ABC和DEF之间的关系是：∠A = ∠D，∠B = ∠E，BC/DE = 4/7。

如果BC = 12，求EF的值。

四、应用题1. 在某个城市的人口数量从2018年的1000万人增长到2020年的1200万人。

求2019年该城市的人口数量，并计算增长的百分比。

2. 一辆汽车以每小时60公里的速度行驶，行驶时间为2小时。

计算该车行驶的距离。

以上是一些IGCSE数学练习题的示例，希望能够帮助您巩固数学知识和应对考试。

建议您在解答这些问题时，尽量使用适当的计算方法和策略，以提高解题效率和准确性。

祝您取得优异的成绩！。

IGCSE math 数学试卷0580_s17_qp_43

This document consists of 19 printed pages and 1 blank page.DC (NH/JG) 130218/2© UCLES 2017[Turn over*0731247115*MATHEMATICS 0580/43Paper 4 (Extended) May/June 20172 hours 30 minutesCandidates answer on the Question Paper.Additional Materials: Electronic calculator Geometrical instrumentsTracing paper (optional).READ THESE INSTRUCTIONS FIRSTWrite your Centre number, candidate number and name on all the work you hand in.Write in dark blue or black pen.You may use an HB pencil for any diagrams or graphs.Do not use staples, paper clips, glue or correction fluid.DO NOT WRITE IN ANY BARCODES.Answer all questions.If working is needed for any question it must be shown below that question.Electronic calculators should be used.If the degree of accuracy is not specified in the question, and if the answer is not exact, give the answer to three significant figures. Give answers in degrees to one decimal place.For π, use either your calculator value or 3.142.At the end of the examination, fasten all your work securely together.The number of marks is given in brackets [ ] at the end of each question or part question.The total of the marks for this paper is 130.Cambridge International ExaminationsCambridge International General Certificate of Secondary Education1 (a) In 2016, a company sold 9600 cars, correct to the nearest hundred.(i) Write down the lower bound for the number of cars sold. (1)(ii) The average profit on each car sold was $2430, correct to the nearest $10.Calculate the lower bound for the total profit.Write down the exact answer.$ (2)(iii) Write your answer to part (a)(ii) correct to 4 significant figures.$ (1)(iv) Write your answer to part (a)(iii) in standard form.$ (1)(b) In April, the number of cars sold was 546.This was an increase of 5% on the number of cars sold in March.Calculate the number of cars sold in March. (3)© UCLES 20170580/43/M/J/17(c) The price of a new car grows exponentially by 3% per year.A new car has a price of $3000 in 2013.Find the price of a new car 4 years later.$ (2)© UCLES 2017[Turn over0580/43/M/J/170580/43/M/J/17© UCLES 20172 (a)y °x °z °DABPQSRCE NOT TO SCALE24°38°PQ is parallel to RS .ABC and ADE are straight lines. Find the values of x , y and z .x = ..................................................y = ..................................................z = ..................................................[3] (b)ABDCNOT TOSCALE42°The points A , B , C and D lie on the circumference of the circle. AB = AD , AC = BC and angle ABD = 42°. Find angle CAB .Angle CAB = (3)(c)NOT TOSCALEThe points P, Q, R and S lie on the circumference of the circle, centre O.Angle QOS =146°.Find angle QRS.QRS = . (2)Angle© UCLES 2017[Turn over0580/43/M/J/170580/43/M/J/17© UCLES 20173The table shows some values for 24y x x 32=+.x –2.2–2–1.5–1–0.500.50.8y–1.940.753.58(a) Complete the table.[4](b) Draw the graph of 24y x x 32=+ for 2.20.8x G G - .[4] (c) Find the number of solutions to the equation 243x x 32+=. (1)0580/43/M/J/17© UCLES 2017[Turn over(d) (i) The equation 241x x x 32+-= can be solved by drawing a straight line on the grid.Write down the equation of this straight line.y = ..................................................[1] (ii) Use your graph to solve the equation 241x x x 32+-=.x = ............................ or x = ............................ or x = ............................[3] (e) The tangent to the graph of 24y x x 32=+ has a negative gradient when x k =. Complete the inequality for k ....................... 1 k 1 . (2)0580/43/M/J/17© UCLES 20174 (a) The diagram shows a solid metal prism with cross section ABCDE .BGFKJ DCAEH2 cm7 cm4 cm8 cm 4 cmNOT TOSCALE(i) Calculate the area of the cross section ABCDE .............................................cm 2 [6](ii) The prism is of length 8 cm.Calculate the volume of the prism.............................................cm 3 [1](b) A cylinder of length 13 cm has volume 280 cm3.(i) Calculate the radius of the cylinder..............................................cm [3] (ii) The cylinder is placed in a box that is a cube of side 14 cm.Calculate the percentage of the volume of the box that is occupied by the cylinder................................................% [3]© UCLES 2017[Turn over0580/43/M/J/175 (a) Haroon has 200 letters to post.The histogram shows information about the masses, m grams, of the letters.Mass (grams)mFrequencydensity(i) Complete the frequency table for the 200 letters.Mass (m grams)0 1m G 1010 1m G 2020 1m G 2525 1m G 3030 1m G 50Frequency5017[3](ii) Calculate an estimate of the mean mass.................................................g [4]0580/43/M/J/17© UCLES 2017(b) Haroon has 15 parcels to post.The table shows information about the sizes of these parcels.Size Small LargeFrequency96Two parcels are selected at random.Find the probability that(i) both parcels are large, (2)(ii) one parcel is small and the other is large. (3)(c) The probability that a parcel arrives late is 803.4000 parcels are posted.Calculate an estimate of the number of parcels expected to arrive late. (1)6(a) Describe fully the single transformation that maps shape A onto(i) shape B,...................................................................................................................................................... (2)(ii) shape C....................................................................................................................................................... (3)(b) Draw the image of shape A after rotation through 90° anticlockwise about the point (3, -1). [2]y=. [2] (c) Draw the image of shape A after reflection in 1f p.(d) Describe fully the single transformation represented by the matrix 3003.............................................................................................................................................................. (3)7 (a) Solve the simultaneous equations. You must show all your working.x y 2311+=x y 3550-=- x = ..................................................y = (4)(b) 12x x a x b 22-+=+^h Find the value of a and the value of b .a = ..................................................b = (3)(c) Write as a single fraction in its simplest form.x x x x 25132-+-+ (4)8 (a)The table shows the marks gained by 10 students in their physics test and their mathematics test.The first six points have been plotted for you.MathematicsmarkPhysics mark[2](ii) What type of correlation is shown in the scatter diagram? (1)(b) The marks of 30 students in a spelling test are shown in the table below.Mark012345Frequency245568Find the mean, median, mode and range of these marks.Mean = ..................................................Median = ..................................................Mode = ..................................................Range = (7)(c) The table shows the marks gained by some students in their English test.Mark 527591Number of students x4511The mean mark for these students is 70.3 .Find the value of x.= (3)x9ACQ B525 m872 m104°NOT TO SCALEABC is a triangular field on horizontal ground. There is a vertical pole BQ at B .AB = 525 m, BC = 872 m and angle ABC = 104°.(a) Use the cosine rule to calculate the distance AC .AC = ..............................................m [4] (b) The angle of elevation of Q from C is 1.0°.Showing all your working, calculate the angle of elevation of Q from A . (4)(c) (i) Calculate the area of the field.............................................. m2 [2] (ii) The field is drawn on a map with the scale 1 : 20 000.Calculate the area of the field on the map in cm2.............................................cm2 [2]10 = {21, 22, 23, 24, 25, 26, 27, 28, 29, 30} A = { x : x is a multiple of 3} B = { x : x is prime} C = { x : x G 25}(a) Complete the Venn diagram.ABCᏱ[4] (b) Use set notation to complete the statements. (i) 26 ..................... B [1](ii) A + B = .....................[1](c) List the elements of B , (C + A ). (2)(d) Find (i) n(C ),...................................................[1] (ii) B B C n ,+l ^^h h ....................................................[1] (e)A C +^h is a subset of A C ,^h . Complete this statement using set notation.A C +^h ..................... A C ,^h [1]11 The table shows the first four terms in sequences A, B, C and D.Complete the table.BLANK PAGEPermission to reproduce items where third-party owned material protected by copyright is included has been sought and cleared where possible. Every reasonable effort has been made by the publisher (UCLES) to trace copyright holders, but if any items requiring clearance have unwittingly been included, the publisher will be pleased to make amends at the earliest possible opportunity.To avoid the issue of disclosure of answer-related information to candidates, all copyright acknowledgements are reproduced online in the Cambridge International Examinations Copyright Acknowledgements Booklet. This is produced for each series of examinations and is freely available to download at after the live examination series.Cambridge International Examinations is part of the Cambridge Assessment Group. Cambridge Assessment is the brand name of University of Cambridge Local Examinations Syndicate (UCLES), which is itself a department of the University of Cambridge.。

igcse additional math题库

IGCSE Additional Math题库随着国际教育的普及，越来越多的学生选择参加IGCSE考试。

IGCSE Additional Math作为其中的一门科目，备受学生们的关注。

为了帮助学生更好地备考，我们整理了一份IGCSE Additional Math题库，供学生参考。

一、代数1. 求解方程组：2x + 3y = 7，3x - 2y = 82. 求解二次方程：x² - 5x + 6 = 03. 计算多项式的值：3x² + 5x - 2，当x = 2时4. 求解不等式：2x + 5 > 135. 解决复合函数：f(g(x))二、几何1. 求解三角形的面积，已知两边长和夹角2. 计算圆的面积和周长3. 求解平行线和垂直线的性质4. 计算多边形内角和5. 解决空间几何问题：体积、表面积等三、微积分1. 求函数的导数和不定积分2. 计算定积分：∫(3x² + 2)dx3. 求解微分方程：dy/dx = 3x² - 44. 解决最值和极值问题5. 计算定积分和面积四、统计1. 计算平均数、中位数和众数2. 分析频数分布表和直方图3. 计算标准差和方差4. 解决概率问题：排列、组合、事件概率等5. 进行抽样调查和统计推断五、概率1. 计算事件的概率与概率分布2. 解决条件概率和独立事件问题3. 根据概率分布表求期望值和方差4. 进行排列和组合问题5. 计算生日问题和齐次概率问题通过这份题库的学习和练习，相信学生们能更全面地掌握IGCSE Additional Math的知识点，为考试取得好成绩打下坚实的基础。

希望学生们能够认真对待每一道题目，通过不断的练习和思考，提高自己的解题能力和应试技巧。

祝愿所有参加IGCSE Additional Math考试的学生都能取得优异的成绩！由于篇幅有限，上述的IGCSE Additional Math题库并不能涵盖所有可能出现的题目类型。

2024 GRE考试专题数学历年真题集锦

2024 GRE考试专题数学历年真题集锦GRE考试是对申请美国研究生院的学生进行综合能力测试的重要方式之一。

其中，数学部分是考生必须重点准备和应对的内容之一。

为了帮助考生更好地备考，本文整理了2024年GRE考试数学部分的历年真题集锦，供考生参考和复习使用。

一、整数和1. 如果a和b都是正整数，且a + b = 8，那么a和b可能的取值有哪些？答案：(1, 7), (2, 6), (3, 5), (4, 4)2. 如果m和n都是正整数，且m - n = 14，那么m和n的最小公倍数是多少？答案：28二、几何1. 一根长为10英尺的绳子被剪成两段，使得其中一段的长度是另外一段的2倍。

求较短一段的长度。

答案：4英尺2. 一个半径为5的圆与一个半径为8的圆相切于一点，并且两个圆的圆心之间的距离为13。

求两个圆相切点之间的距离。

答案：12三、代数1. 如果a是一个正整数，并且a^2 - 5a + 6 = 0，那么a的值是多少？答案：2或32. 设a、b、c是正整数，且满足a + b = c。

若a能被5整除，c能被9整除，那么b能被几整除？答案：4四、概率与统计1. 在一个有10个数字的集合中，每一个数字都是从1到10中随机选取的，那么从这个集合中选取一个数字，并且这个数字是偶数的概率是多少？答案：1/22. 一张标准扑克牌中红桃的数量为13，黑桃的数量为13。

从扑克牌中随机抽取一张牌，那么这张牌为红桃或黑桃的概率是多少？答案：26/52 = 1/2通过以上历年真题的集锦，考生可以更好地理解GRE数学部分的题目类型和解题思路。

每个题目的解答都提供了详细的答案，考生可以通过演算来验证答案的正确性，并进行自我评估。

为了顺利完成GRE考试，考生需要做到以下几点：首先，掌握基础知识。

GRE数学部分主要考察考生对数学基本概念和方法的理解和运用能力。

因此，考生需要熟悉数学基本概念和公式，同时要能够快速准确地运用这些概念和公式解题。

ALEVEL IGCSE 数学试卷-1汇编

• Note:
B2 or A2 means that the candidate can earn 2 or 0. B2/1/0 means that the candidate can earn anything from 0 to 2.
The marks indicated in the scheme may not be subdivided. If there is genuine doubt whether a candidate has earned a mark, allow the candidate the benefit of the doubt. Unless otherwise indicated, marks once gained cannot subsequently be lost, e.g. wrong working following a correct form of answer is ignored.
A Accuracy mark, awarded for a correct answer or intermediate step correctly obtained. Accuracy marks cannot be given unless the associated method mark is earned (or implied).
CAMBRIDGE INTERNATIONAL EXAMINATIONS Cambridge International Advanced Subsidiary and Advanced Level
MARK SCHEME for the May/June 2015 series

accurate)

【独家带详解答案】IGCSE2019年数学真题卷1(060612)_20200830123606

Candidates answer on the Question Paper. Additional Materials: Electronic calculator
0606/12 May/June 2019
2 hours
READ THESE INSTRUCTIONS FIRST
Write your centre number, candidate number and name on all the work you hand in. Write in dark blue or black pen. You may use an HB pencil for any diagrams or graphs. Do not use staples, paper clips, glue or correction fluid. DO NOT WRITE IN ANY BARCODES.
At the end of the examination, fasten all your work securely together. The number of marks is given in brackets [ ] at the end of each question or part question. The total number of marks for this paper is 80.
*0773973091*
Cambridge Assessment International Education Cambridge International General Certificate of Secondary Education
ADDITIONAL MATHEMATICS Paper 1

剑桥IGCSE课程数学科目试卷四

UCLES 2007
Answer(c)
0607/04/SP/10
minutes [3] [Turn over
4 2 f(x) = 5 .
1− x (a) Find f(–9).
For Examiner's
Use
(b) Solve f(x) = 2.
Answer(a)
READ THESE INSTRUCTIONS FIRST
Write your Centre number, candidate number and name on all the work you hand in. Write in dark blue or black pen. Do not use staples, paper clips, highlighters, glue or correction fluid. You may use a pencil for any diagrams or graphs.
For Examiner's
Use
Answer(a)
km/h [2]
(b) One day, the train departed at 08 50 but, due to delays, the average speed was reduced by 10%. Calculate (i) the new arrival time,
UNIVERSITY OF CAMBRIDGE INTERNATIONAL EXAMINATIONS International General Certificate of Secondary Education
CAMBRIDGE INTERNATIONAL MATHEMATICS Paper 4 (Extended) SPECIMEN PAPER

igcse考试题0580_w02_qp_1

Paris
Answer.……………….……… [3]
15 (a) Write down the values of 20 = ……. , 21 = ……. , 22 = ……. , 23 = ……. , 24 = ……. [2]
(b)
Change
5 49
to a decimal. Write down your full calculator display.
4
10 Complete this diagram accurately so that it has rotational symmetry of order 3 about the point O.
For Examiner's
Use
O
[2]
11 An athlete’s time for a race was 43 .78 seconds.
–3 £ n < 3.
List all the possible values of n.
Answer.……………….……… [2]
6 B
54° A
NOT TO SCALE
AB and AC are tangents to the circle,
centre O.
O
Angle BAC = 54°.
(a) Write down the size of angle ABO.
OCTOBER/NOVEMBER SESSION 2002
Candidates answer on the question paper. Additional materials:
Electronic calculator Geometrical instruments Mathematical tables (optional) Tracing paper (optional)

0606_m19_qp_22IGCSE数学真题)2019年3月

1. ALGEBRA
x = −b
b2 − 4ac 2a
Binomial Theorem
( ) ( ) ( ) (a + b)n = an +
n 1
an–1 b +
n 2
an–2
b2
+
…
+
n r
an–r
br
+
…
+
bn,
( ) where n is a positive integer and
n r
=
(n
∆
=
1 2
bc
sin
A
© UCLES 2019
0606/22/F/M/19
3
1 A band can play 25 different pieces of music. From these pieces of music, 8 are to be selected for a concert.
DC (KS/TP) 165271/2 © UCLES 2019
This document consists of 14 printed pages and 2 blank pages.
[Turn over
2 Mathematical Formulae
Quadratic Equation For the equation ax2 + bx + c = 0,
*1438509375*
Cambridge Assessment International Education Cambridge International General Certificate of Secondary Education

【最新】igcse试卷-精选word文档 (16页)

本文部分内容来自网络整理，本司不为其真实性负责，如有异议或侵权请及时联系，本司将立即删除！== 本文为word格式，下载后可方便编辑和修改！ ==igcse试卷篇一：201X年IGCSE数学考试题1. Solve the equation(9)cos x + ?(1 ? sin 2x) = 0, in the interval 0? ? x < 360?.12(1?x)2. (a) For the binomial expansion of , ?x? < 1, in ascending powers of x,(i) find the first four terms,(ii) write down the coefficient of xn.(2)?xnnx?2(1?x)n?1(b) Hence, show that, for ?x? < 1, = .(2)(a?1)x?x2(an?1)x??2(1?x)n?1(c) Prove that, for ?x? < 1, , where a is a constant.(4)?5n?1?3nn?12(d) Hence evaluate .(2)3. f(x) = x3 ? (k + 4)x + 2k, where k is a constant.(a) Show that, for all values of k, the curve with equation y = f(x) passes through the point (2, 0).(1)(b) Find the values of k for which the equation f(x) = 0 has exactly two distinct roots.(5)Given that k > 0, that the x-axis is a tangent to the curve with equation y = f(x), and that the line y = p intersects the curve in three distinct points,(c) find the set of values that p can take. (5) ?n4.y(0, 4)The circle, with centre C and radius r, touches the y-axis at (0, 4) and also touches the line with equation 4y ? 3x = 0, as shown in Fig.1.(a) (i) Find the value of r.(8)(b)(4)31??(ii) Show that arctan + 2 acrtan = 1 Figure 1 ?. The line with equation 4x + 3y = q, q > 12, is a tangent to the circle. Find the value of q. 1dy2?(1?t). dt5. (a) Given that y = ln [t + ?(1 +t2)],show that =(3)The curve C has parametric equations12?(1?t), y = ln [t + ?(1 + t2)], t ? ?. x = A student was asked to prove that, for t > 0, the gradient of the tangent to C is negative. The attempted proof was as follows:?1??t??y = ln ?x??tx?1???x??= ln= ln (tx + 1) ?ln xdy? dx tx?11xt?x = t1?= tx?1xt?(1?t2)(1?t2)t??(1?t2)= – = t??(1?t2) ? ? (1 + t2)dyAs (1 + t2) > 0, and t + ?(1 + t2) > 0 for t > 0, dx < 0 for t > 0.(b) (i) Identify the error in this attempt.(ii) Give a correct version of the proof.(6)(c) Prove that ln [?t + ?(1 + t2)] = ?ln [t + ?(1 + t2)].(3)(d) Deduce that C is symmetric about the x-axis and sketch the graph of C.(3)6. f(x) = x ? [x], x ? 0where [x] is the largest integer ? x.For example, f(3.7) = 3.7 ? 3 = 0.7; f(3) = 3 ? 3 = 0.(a) Sketch the graph of y = f(x) for 0 ? x < 4.(3)??f(x)dx(b) Find the value of p for which ?2= 0.18.(3)Given that 1g(x) = 1?kx,x ? 0,k > 0,and that x0 = is a root of the equation f(x) = g(x),(c) find the value of k. (2)p2(1)The root of f(x) = g(x) in the interval n < x < n + 1 is xn, where n is an integer.(e) Prove that2xn2 ? (2n ? 1)xn ? (n + 1) = 0. (d) Add a sketch of the graph of y = g(x) to your answer to part (a).(4)7. Triangle ABC, with BC = a, AC = b and AB = c is inscribed in a circle. Given that AB is a diameter of the circle and that a2, b2 and c2 are three consecutive terms of an arithmetic progression (arithmetic series),(a) express b and c in terms of a,(4)。

IGCSE数学

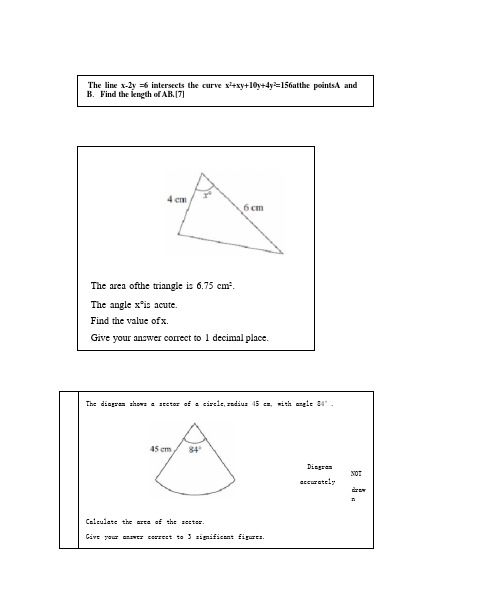
The line x-2y=6intersects the curve x²+xy+10y+4y²=156atthe pointsA and B. Find the length of AB.[7]The area ofthe triangle is 6.75 cm².The angle x°is acute.Find the value of x.Give your answer correct to 1 decimal place.The diagram shows a sector of a circle,radius 45 cm, with angle 84°.DiagramNOTaccuratelydrawn Calculate the area of the sector.Give your answer correct to 3 significant figures.B110°30°Diagram NOT accurately drawnCalculate the length of AC.Give your answer correct to 3 significant figures.3.4 cm叮叮小文库A cone has slant height 4 cm and base radius r cm.+rcm →The total surface area of the cone is Calculate the value of r.Diagram NOTaccurately drawnNOT TOSCALEA 8.3 cm BX 5.5cm20°叮叮小文库In the diagram the lines AB and CD are parallel.The lines AD and BC intersect at X.Angle XDC=35°and angle CXD=120°.(a)(i) Write down the size of angle BAX.Answer(a(i)Angle BAX= (1)(il) Write down the size of angle ABX.Answer(a)(ii)Angle ABX= (1)(b) Complete the statementTriangle AXB is..............……to triangle DXC.[1](c) AB=8.3cm,BX=5.5cm and CD=16.6cm.Calculate the length of CX.C120mNOT TO SCAL EIn quadrilateral ABCD,AB=77m,BC=120m,CD=60m and diagonalAC=55m. Angle CAD=45°,angle BAC=x°and angle ADC=y°(a) Calculate the value of x.(b) Calculate the value of y. (c) The bearing of D from A is090°. Find the bearing of(i) A from C, (ii) B from A.4.9 cm3.6 cm 2.4E2.8 cmCBDiagram NOT accurately drawnB60m7x° 45°导yD 4cmA,B,C and D are four points on the circumference of a circle. The chords AC and BD intersect at E.AE=3.6cm,CE=2.8cm,DE=2.4cm andAD=4.9cm.(a' Calculate the length of BE.(b'Calculate the size of angleAED.Give your answer correct to 3 significant figures.CNOTTOSCALED11.1cm3770°EUTS44° Diagram NOT P accurately drawnL08°QRQ. R,sand Tare points on the circumference of a circle.PU is a tangent to the circle at T.PQr is a straight line.Angle PQT =L08°Angle STR=44°.Work out the size of angle srv.You must give a reason for each step in your working.Find the values of k for which the line y=k-6x is a tangent to the curve y=x(2x+k).。