数学专业英语概述

合集下载

数学专业英语X资料

formulating these theorems 公式化这些定理
be similar to 和什么相似
作为讨论和、积等的极限的基本规则对于收敛序列的极限也是成立的。读者自己公式化这些定理应该不会有困难，它们的证明有点类似于3.5节中给出的那些证明.
divergent sequence 发散序列
术语“收敛序列”通常仅指极限为有限的序列，具有无限极限的序列称为发散。当然存在没有无限极限的发散序列，有下列公式定义的序列，就是例子f(n)=(1)ⁿ,f(n)=sin(nￗ/2),f(n)=(-1)ⁿ(1+1/n),f(n)=e(nￗi/2).
The basic rules for dealing with limits of sums ,products ,etc., also hold for limits of convergent sequence.The reader should have no difficulty in formulating these theorems for himself. Their proofs are somewhat similar to those given in Section 3.5.
show that the relation f(n)→L implies v(n)→a
and u(n)→b as n→∝.Conversely ,the inequality
|f(n)-L|≦|u(n)-a|+|v(n)-b| shows that the two
relations u(n)→a and v(n)→b imply f(n)→L as
数学专业英语
信息151 肖猛王祎
二：The limit of a sequence

数学专业英语-21页精选文档

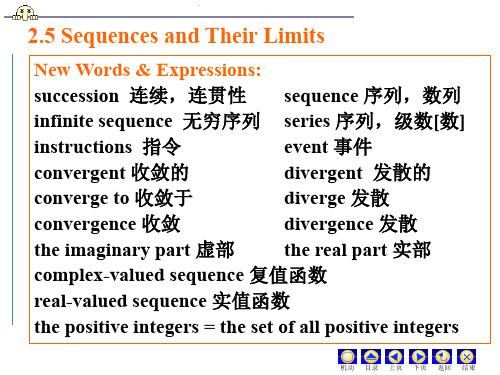
the positive integers = the set of all positive integers
机动目录上页下页返回结束
In everyday usage of the English language, the words “sequence” and “series” are synonyms, and they are used to suggest a succession of things or events arranged in some order. 在日常英语中，单词“sequence” 和 “series” 是同义词，用以表示按某种次序排列的一串东西或事件。
A function f whose domain is the set of all positive integers 1, 2, 3, … is called an infinite sequence. The function value f(n) is called the nth term of the sequence. 以正整数集为定义域的函数称为序列。…
机动目录上页下页返回结束
A sequence { an } is said to have a limit L if, for every
positive number number N ( which may depend on e ) such that
机动目录上页下页返回结束
Very often the dependence on n is denoted by using subscripts, and we write an , xn . 序列各项对 n 的相关性常利用下标来表示，写成 an , xn 。

数学专业英语2-2

A line segment is named by the two end points. When no confusion result, the expression “line segment AB” is often replaced by segment AB or, simply, line AB.
学生通过几何的学习而达到的最主要目标是：在听，读，和思考时变得更加审慎。在学习几何的过程中，他们不再盲目地接受一些陈述和思想，而是在得出结论之前学会了清楚和审慎的思考。
2－B Some geometrical terms
1. Solids and planes. A solid is a three-dimensional figure. Common examples of solids are cube, sphere, cylinder, cone and pyramid.
点没有长度，宽度和厚度，但是标记了一个位置。我们熟悉铅笔尖，针尖这样的表达。我们可以用一个小圆点来表示一个点，在它旁边用打印体大写字母来命名，如图2-2-1中的点A。
The line is named by labeling two points on it with capital letters or one small letter near it. The straight line extends infinitely far in two directions and has no ends. The part of the line between two points on the line is termed a line segment.
有三种线：直线，折线和曲线。曲线是指其中没有任何部分是直的。折线是由连起来的直线段构成，如图2-2-3中的ABCDE.

数学专业英语(Doc版).20

数学专业英语－Sequences and SeriesSeries are a natural continuation of our study of functions. In the previous cha pter we found howto approximate our elementary functions by polynomials, with a certain error te rm. Conversely, one can define arbitrary functions by giving a series for them. We shall see how in the sections below.In practice, very few tests are used to determine convergence of series. Esse ntially, the comparision test is the most frequent. Furthermore, the most import ant series are those which converge absolutely. Thus we shall put greater emp hasis on these.Convergent SeriesSuppose that we are given a sequcnce of numbersa1,a2,a3…i.e. we are given a number a n, for each integer ｎ＞1.We form the sumsS n=a1+a2+…+a nIt would be meaningless to form an infinite suma1+a2+a3+…because we do not know how to add infinitely many numbers. However, if ou r sums S n approach a limit as n becomes large, then we say that the sum of our sequence converges, and we now define its sum to be that limit.The symbols∑a=1 ∞a nwill be called a series. We shall say that the series converges if the sums app roach a limit as n becomes large. Otherwise, we say that it does not converge, or diverges. If the seriers converges, we say that the value of the series is∑a=1∞=lim a→∞S n=lim a→∞(a1+a2+…+a n)In view of the fact that the limit of a sum is the sum of the limits, and other standard properties of limits, we get:THEOREM 1. Let{ a n}and { b n}(n=1,2,…)be two sequences and assume that the series∑a=1∞a n∑a=1∞b nconverge. Then ∑a=1∞(a n + b n ) also converges, and is equal to the sum of the two series. If c is a number, then∑a=1∞c a n=c∑a=1∞a nFinally, if s n=a1+a2+…+a n and t n=b1+b2+…+b n then∑a=1∞a n ∑a=1∞b n=lim a→∞s n t nIn particular, series can be added term by term. Of course , they cannot be multiplied term by term.We also observe that a similar theorem holds for the difference of two serie s.If a series ∑a n converges, then the numbers a n must approach 0 as n beco mes large. However, there are examples of sequences {an} for which the serie s does not converge, and yet lim a→∞a n=0Series with Positive TermsThroughout this section, we shall assume that our numbers a n are ＞0. Then t he partial sumsS n=a1+a2+…+a nare increasing, i.e.s1＜s2 ＜s3＜…＜s n＜s n+1＜…If they are approach a limit at all, they cannot become arbitrarily large. Thus i n that case there is a number B such thatS n< Bfor all n. The collection of numbers {s n} has therefore a least upper bound ,i.e. there is a smallest number S such thats n<Sfor all n. In that case , the partial sums s n approach S as a limit. In other wo rds, given any positive number ε>0, we haveS –ε< s n < Sfor all n .sufficiently large. This simply expresses the fact that S is the least o f all upper bounds for our collection of numbers s n. We express this as a theo rem.THEOREM 2. Let{a n}(n=1,2,…)be a sequence of numbers>0 and letS n=a1+a2+…+a nIf the sequence of numbers {s n} is bounded, then it approaches a limit S , wh ich is its least upper bound.Theorem 3 gives us a very useful criterion to determine when a series with po sitive terms converges:THEOREM 3. Let∑a=1∞a n and∑a=1∞b n be two series , with a n>0 for all n an d b n>0 for all n. Assume that there is a number c such thata n< cb nfor all n, and that∑a=1∞b n converges. Then ∑a=1∞a n converges, and∑a=1∞a n ≤c∑a=1∞b nPROOF. We havea1+…+a n≤cb1+…+cb n=c(b1+…+b n)≤c∑a=1∞b nThis means that c∑a=1∞b n is a bound for the partial sums a1+…+a n.The least u pper bound of these sums is therefore ≤c∑a=1∞b n, thereby proving our theore m.Differentiation and Intergration of Power Series.If we have a polynomiala0+a1x+…+a n x nwith numbers a0,a1,…,a n as coefficients, then we know how to find its derivati ve. It is a1+2a2x+…+na n x n–1. We would like to say that the derivative of a ser ies can be taken in the same way, and that the derivative converges whenever the series does.THEOREM 4. Let r be a number >0 and let ∑a n x n be a series which conv erges absolutely for ∣x∣<r. Then the series ∑na n x n-1also converges absolutel y for∣x∣<r.A similar result holds for integration, but trivially. Indeed, if we have a series ∑a=1∞a n x n which converges absolutely for ∣x∣<r, then the series∑a=1∞a n/n+1 x n+1=x∑a=1∞a n x n∕n+1has terms whose absolute value is smaller than in the original series.The preceding result can be expressed by saying that an absolutely converge nt series can be integrated and differentiated term by term and and still yields an absolutely convergent power series.It is natural to expect that iff (x)=∑a=1∞a n x n,then f is differentiable and its derivative is given by differentiating the series t erm by term. The next theorem proves this.THEOREM 5. Letf (x)=∑a=1∞a n x nbe a power series, which converges absolutely for∣x∣<r. Then f is differentia ble for ∣x∣<r, andf′(x)=∑a=1∞na n x n-1.THEOREM 6. Let f (x)=∑a=1∞a n x n be a power series, which converges abso lutely for ∣x∣<r. Then the relation∫f (x)d x=∑a=1∞a n x n+1∕n+1is valid in the interval ∣x∣<r.We omit the proofs of theorems 4,5 and 6.Vocabularysequence 序列positive term 正项series 级数alternate term 交错项approximate 逼近,近似 partial sum 部分和elementary functions 初等函数 criterion 判别准则(单数)section 章节 criteria 判别准则(多数)convergence 收敛(名词) power series 幂级数convergent 收敛(形容词) coefficient 系数absolute convergence 绝对收敛 Cauchy sequence 哥西序列diverge 发散radius of convergence 收敛半径term by term 逐项M-test M—判别法Notes1. series一词的单数和复数形式都是同一个字.例如:One can define arbitrary functions by giving a series for them(单数)The most important series are those which converge absolutely(复数)2. In view of the fact that the limit of a sum of the limits, and other standard properties of limits, we get:Theorem 1…这是叙述定理的一种方式: 即先将事实说明在前面,再引出定理. 此句用in view of the fact that 说明事实,再用we get 引出定理.3. We express this as a theorem.这是当需要证明的事实已再前面作了说明或加以证明后,欲吧已证明的事实总结成定理时,常用倒的一个句子,类似的句子还有(参看附录Ⅲ):We summarize this as the following theorem; Thus we come to the following theorem等等.4. The least upper bound of these sums is therefore ≤c∑a=1∞b n, thereby proving our theorem.最一般的定理证明格式是”给出定理…定理证明…定理证毕”,即thereby proving our theorem;或we have thus proves the theorem或This completes the proof等等作结尾(参看附录Ⅲ).5. 本课文使用较多插入语.数学上常见的插入语有:conversely; in practice; essentially; in particular; ind eed; in other words; in short; generally speaking 等等.插入语通常与句中其它成份没有语法上的关系,一般用逗号与句子隔开,用来表示说话者对句子所表达的意思的态度.插入语可以是一个词,一个短语或者一个句子.ExerciseⅠ. Translate the following exercises into Chinese:1. In exercise 1 through 4,a sequence f (n) is defined by the formula given. In each case, (ⅰ)Determine whether the sequence (the formulae are omitted).2. Assume f is a non–negative function defined for all x>1. Use the methodsuggested by the proof of the integral test to show that∑k=1n-1f(k)≤∫1n f(x)d x ≤∑k=2n f(k)Take f(x)=log x and deduce the inequalitiesc•n n•c-n< n！<c•n n+1•c-nⅡ. The proof of theorem 4 is given in English as follows(Read the proof through and try to learn how a theorem is proved, then translate this proof into Chinese ):Proof of theorem 4 Since we are interested in the absolute convergence. We may assume that a n>0 for all n. Let 0<x<r, and let c be a number such that x<c<r. Recall that lim a→∞n1/n=1.We may write n a n x n =a n(n1/n x)n. Then for all n sufficiently large, we conclude that n1/n x<c. This is because n1/n comes arbitrarily close to x and x<c. Hence for all n sufficiently large, we have na n x n<a n c n. We can then compare the series ∑nax n with∑a n c n to conclude that∑na n x n converges. Since∑na n x n-1=1n/x∑na n x n, we have proved theorem 4.Ⅲ. Recall from what you have learned in Calculus about (ⅰ) Cauchy sequence and (ⅱ) the radius of c onvergence of a power series.Now give the definitions of these two terms respectively.Ⅳ. Translate the following sentences into Chinese:1. 一旦我们能证明,幂级数∑a n z n在点z=z1收敛,则容易证明,对每一z1∣z∣<∣z1∣,级数绝对收敛;2. 因为∑a n z n在z=z1收敛,于是,由weierstrass的M—判别法可立即得到∑a n z n在点z,∣z∣<z1的绝对收敛性;3. 我们知道有限项和中各项可以重新安排而不影响和的值,但对于无穷级数,上述结论却不总是真的。

数学专业英语(Doc版).Word5

数学专业英语－Differential CalculusHistorical IntroductionNewton and Leibniz,quite independently of one another,were largely responsible for developing the ideas of integral calculus to the point where hitherto insur mountable problems could be solved by more or less routine methods.The succ essful accomplishments of these men were primarily due to the fact that they were able to fuse together the integral calculus with the second main branch o f calculus,differential calculus.The central idea of differential calculus is the notion of derivative.Like the inte gral,the derivative originated from a problem in geometry—the problem of find ing the tangent line at a point of a curve.Unlile the integral,however,the deriva tive evolved very late in the history of mathematics.The concept was not form ulated until early in the 17th century when the French mathematician Pierre de Fermat,attempted to determine the maxima and minima of certain special func tions.Fermat’s idea,basically very simple,can be understood if we refer to a curve a nd assume that at each of its points this curve has a definite direction that ca n be described by a tangent line.Fermat noticed that at certain points where th e curve has a maximum or minimum,the tangent line must be horizontal.Thus t he problem of locating such extreme values is seen to depend on the solution of another problem,that of locating the horizontal tangents.This raises the more general question of determining the direction of the tange nt line at an arbitrary point of the curve.It was the attempt to solve this gener al problem that led Fermat to discover some of the rudimentary ideas underlyi ng the notion of derivative.At first sight there seems to be no connection whatever between the problem of finding the area of a region lying under a curve and the problem of findin g the tangent line at a point of a curve.The first person to realize that these t wo seemingly remote ideas are,in fact, rather intimately related appears to have been Newton’s teacher,Isaac Barrow(1630-1677).However,Newton and Leibniz were the first to understand the real importance of this relation and they explo ited it to the fullest,thus inaugurating an unprecedented era in the development of mathematics.Although the derivative was originally formulated to study the problem of tang ents,it was soon found that it also provides a way to calculate velocity and,mo re generally,the rate of change of a function.In the next section we shall consi der a special problem involving the calculation of a velocity.The solution of this problem contains all the essential fcatures of the derivative concept and may help to motivate the general definition of derivative which is given below.A Problem Involving VelocitySuppose a projectile is fired straight up from the ground with initial velocity o f 144 feet persecond.Neglect friction,and assume the projectile is influenced onl y by gravity so that it moves up and back along a straight line.Let f(t) denote the height in feet that the projectile attains t seconds after firing.If the force of gravity were not acting on it,the projectile would continue to move upward with a constant velocity,traveling a distance of 144 feet every second,and at ti me t we woule have f(t)=144 t.In actual practice,gravity causes the projectile t o slow down until its velocity decreases to zero and then it drops back to eart h.Physical experiments suggest that as the projectile is aloft,its height f(t) is gi ven by the formula(1)f(t)=144t –16 t2The term –16t2is due to the influence of gravity.Note that f(t)=0 when t=0 a nd when t=9.This means that the projectile returns to earth after 9 seconds and it is to be understood that formula (1) is valid only for 0<t<9.The problem we wish to consider is this:To determine the velocity of the proj ectile at each instant of its motion.Before we can understand this problem,we must decide on what is meant by the velocity at each instant.To do this,we int roduce first the notion of average velocity during a time interval,say from time t to time t+h.This is defined to be the quotient.Change in distance during time interval =f(t+h)-f(t)/hThis quotient,called a difference quotient,is a number which may be calculated whenever both t and t+h are in the interval[0,9].The number h may be positiv e or negative,but not zero.We shall keep t fixed and see what happens to the difference quotient as we take values of h with smaller and smaller absolute v alue.The limit process by which v(t) is obtained from the difference quotient is wri tten symbolically as follows:V(t)=lim(h→0)[f(t+h)-f(t)]/hThe equation is used to define velocity not only for this particular example bu t,more generally,for any particle moving along a straight line,provided the position function f is such that the differerce quotient tends to a definite limit as h approaches zero.The example describe in the foregoing section points the way to the introducti on of the concept of derivative.We begin with a function f defined at least on some open interval(a,b) on the x axis.Then we choose a fixed point in this in terval and introduce the difference quotient[f(x+h)-f(x)]/hwhere the number h,which may be positive or negative(but not zero),is such th at x+h also lies in(a,b).The numerator of this quotient measures the change in the function when x changes from x to x+h.The quotient itself is referred to a s the average rate of change of f in the interval joining x to x+h.Now we let h approach zero and see what happens to this quotient.If the quot ient.If the quotient approaches some definite values as a limit(which implies th at the limit is the same whether h approaches zero through positive values or through negative values),then this limit is called the derivative of f at x and is denoted by the symbol f’(x) (read as “f prime of x”).Thus the formal defi nition of f’(x) may be stated as follows:Definition of derivative.The derivative f’(x)is defined by the equationf’(x)=lim(h→o)[f(x+h)-f(x)]/hprovided the limit exists.The number f’(x) is also called the rate of change of f at x.In general,the limit process which produces f’(x) from f(x) gives a way of ob taining a new function f’from a given function f.This process is called differ entiation,and f’is called the first derivative of f.If f’,in turn,is defined on an interval,we can try to compute its first derivative,denoted by f’’,and is calle d the second derivative of f.Similarly,the nth derivative of f denoted by f^(n),is defined to be the first derivative of f^(n-1).We make the convention that f^(0) =f,that is,the zeroth derivative is the function itself.Vocabularydifferential calculus微积分differentiable可微的intergral calculus 积分学differentiate 求微分hither to 迄今 integration 积分法insurmountable 不能超越 integral 积分routine 惯常的integrable 可积的fuse 融合integrate 求积分originate 起源于sign-preserving保号evolve 发展，引出 axis 轴（单数）tangent line 切线 axes 轴（复数）direction 方向 contradict 矛盾horizontal 水平的contradiction 矛盾vertical 垂直的 contrary 相反的rudimentary 初步的，未成熟的composite function 合成函数，复合函数area 面积composition 复合函数intimately 紧密地interior 内部exploit 开拓，开发 interior point 内点inaugurate 开始 imply 推出，蕴含projectile 弹丸 aloft 高入云霄friction摩擦initial 初始的gravity 引力 instant 瞬时rate of change 变化率integration by parts分部积分attain 达到definite integral 定积分defferential 微分indefinite integral 不定积分differentiation 微分法 average 平均Notes1. Newton and Leibniz,quite independently of one another,were largely responsible for developing…by more or less routine methods.意思是：在很大程度上是牛顿和莱伯尼，他们相互独立地把积分学的思想发展到这样一种程度，使得迄今一些难于超越的问题可以或多或少地用通常的方法加以解决。

数学专业英语

数学专业英语数学，作为一门自然科学，对于任何一个国家和民族来说都具有非常重要的意义。

作为一种研究物质与现象本质的学科，数学通过一系列公理和推理，进行精确的描述和分析，从而刻画出了丰富的数学结构和表现形式，成为自然科学中应用最广泛的基础学科之一。

为了更好地传承和发展数学学科，我国数学教育体系逐渐完善，大量优秀的数学教师和研究人员涌现出来，为数学事业不断贡献力量。

同时，我国的数学研究不仅在量上有了大幅度的提升，更在质上得到了越来越多的认可和尊重。

在数学研究领域，英语作为国际性的语言，具有不可替代的地位。

数学专业英语，是由数学领域专业人士使用的一种专门的术语和表达方式。

正如同其他学科一样，数学专业英语也有其独特的语言规范、术语和语法，并且赋予了数学的某些具体含义。

因此，掌握数学专业英语，不仅是进入国际学术圈的重要标志，同时也是增进数学研究深度和广度的关键所在。

为此，本文将从数学专业英语的基本要素、相关研究和未来发展趋势等方面进行探讨和解析。

一、数学专业英语的基本要素1. 语法语法是数学专业英语的基本要素之一，它是制定语言规范的重要基础。

英语语法主要包括名词、动词、形容词和副词等四个基本部分。

而在数学专业英语中，这些基本部分的构成都会涉及到特定的数学概念和符号。

此外，数学专业英语还包括数学符号、公式等。

例如，对于一个数学论文中的一个公式，“f(x)=ax+b”，这个公式中的"f(x)"，代表一个函数；"a"和"b"分别代表函数的系数；"x"则代表自变量。

在这个公式中，如果说我们把"f(x)"看作数学专业英语中的名词，那么"a"和"b"就是形容词，而"x"可以看作动词。

因此，理解数学专业英语中的语法结构和语法规则，对于正确地理解和应用数学专业英语极为重要。

数学专业英语第一章

B(a,r), we have h(x)d (x) h(a) . B
特点二：科学内容的完整性与表达形式的精炼性要求
1、长句较多 2、非限定动词使用频率高
Eg1: By introducing a new smoothing function, the problem is approximated by a family of parameterized optimization problems with twice continuously differentiable objective functions.
3、能用英语书写文章摘要、学术会议通知、学术交流信件等。同时培养简单的英语会话能力。 4、为部分优秀学生攻读研究生奠定数学专业英语的基础，同时让大部分同学了解数学专业英语与生活英语的区别，为今后走上工作岗位，特别是服务于IT业或外资企业有独当一面的能力。
本课程分四部分讲解：
精选课文：1、理解数学专业文章和一般英语文章写作的不同；
对于学习数学的学生和准备从事数学研究的人员在掌握了公共英语的基本知识的基础上如果希望较快地掌握阅读英文版数学教程和科研资料的基本方法进一步学习数学专业英语是必须的
数学专业英语第一讲
为什么要学习数学专业英语？
❖ 数学学科发展需要：20世纪90年代计算机科学技术的迅速发展宣告了人类信息时代的到来。数学，这个古老而又优雅的学科获得了新的发展动力和发挥作用的舞台。
Eg2: A right angle is a 90 angle. 见P31
2. 被动语态出现频率高，应用范围广
Eg1:The Fermat Conjecture has been proved to be true.
Eg2: The function idea may be illustrated schematically in many ways. 见P54

数学专业英语(Doc版).11

数学专业英语－Linear AlgebraFor the definition that follows we assume that we are given a particular field K. The scalars to be used are to be elements of K.DEFINITION. A vector space is a set V of elements called vectors satisfyi ng the following axioms.(A) To every pair, x and y ,of vectors in V corresponds a vector x+y,call ed the sum of x and y, in such a way that.(1) addition is commutative, x + y = y + x.(2) addition is associative, x + ( y + z ) = ( x + y ) + z.(3) there exists in V a unique vector 0 (called the origin ) such that x + 0 = x for every vector x , and(4) to every vector x in V there corresponds a unique vector - x such that x + ( - x ) = 0.(B) To every pair,αand x , where αis a scalar and x is a vector in V ,the re corresponds a vector αx in V , called the product of αand x , in such a way that(1) multiplication by scalars is associative,α(βx ) = (αβ) x(2) 1 x = x for every vector x.(C) (1) multiplication by scalars is distributive with respect to vector addition,α( x + y ) = αx+βy , and(2)multiplication by vectors is distributive with respect to scalar addition,(α+β) x = αx + βx .The relation between a vector space V and the underlying field K is usually d escribed by saying that V is a vector space over K . The associated field of s calars is usually either the real numbers R or the complex numbers C . If V i s linear space and M真包含于V , and if αu -v belong to M for every u an d v in M and every α∈ K , then M is linear subspace of V . If U = { u 1,u 2,…} is a collection of points in a linear space V , then the (linear) span of the set U is the set of all points o the form ∑c i u i, where c i∈ K ,and all but a finite number of the scalars c i are 0.The span of U is al ways a linear subspace of V.A key concept in linear algebra is independence. A finite set { u 1,u 2,…, u} is said to be linearly independent in V if the only way to write 0 = ∑kc i u i is by choosing all the c i= 0 . An infinite set is linearly independent if every finite set is independent . If a set is not independent, it is linearlyd ependent, and in this case, some point in the set can be written as a linear co mbination of other points in the set. A basis for a linear space M is an indep endent set that spans M . A space M is finite-dimensional if it can be spanne d by a finite set; it can then be shown that every spanning set contains a basi s, and every basis for M has the same number of points in it. This common number is called the dimension of M .Another key concept is that of linear transformation. If V and W are linear sp aces with the same scalar field K , a mapping L from V into W is called lin ear if L (u + v ) = L( u ) + L ( v ) and L ( αu ) = αL ( u ) for ever y u and v in V and αin K . With any I , are associated two special linear spaces:ker ( L ) = null space of L = L-1 (0)= { all x ∈V such that L ( X ) = 0 }Im ( L ) = image of L = L( V ) = { all L( x ) for x∈V }.Then r = dimension of Im ( L ) is called the rank of L. If W also has dime nsion n, then the following useful criterion results: L is 1-to-1 if and only if L is onto.In particular, if L is a linear map of V into itself, and the only solu tion of L( x ) = 0 is 0, then L IS onto and is therefore an isomorphism of V onto V , and has an inverse L -1. Such a transformation V is also said to b e nonsingular.Suppose now that L is a linear transformation from V into W where dim ( V ) = n and dim ( W ) = m . Choose a basis {υ 1 ,υ 2 ,…,υn} for V and a basis {w 1 ,w2 ,…,w m} for W . Then these define isomorphisms of V onto K n and W onto K m, respectively, and these in turn induce a linear transfor mation A between these. Any linear transformation ( such as A ) between K n and K m is described by means of a matrix ( a), according to the formula Aij( x ) = y , where x = { x1, x 2,…, x n} y = { y1, y 2,…, y m} and Y j =Σn j=i a ij x i I=1,2,…,m.The matrix A is said to represent the transformation L and to be the represent ation induced by the particular basis chosen for V and W .If S and T are linear transformations of V into itself, so is the compositic tra nsformation ST . If we choose a basis in V , and use this to obtain matrix re presentations for these, with A representing S and B representing T , then ST must have a matrix representation C . This is defined to be the product AB o f the matrixes A and B , and leads to the standard formula for matrix multipli cation.The least satisfactory aspect of linear algebra is still the theory of determinants even though this is the most ancient portion of the theory, dating back to Lei bniz if not to early China. One standard approach to determinants is to regard an n -by- n matrix as an ordered array of vectors( u 1 , u 2,…, u n) and t hen its determinant det ( A ) as a function F( u 1 , u 2 ,…, u n) of these n vectors which obeys certain rules.The determinant of such an array A turns out to be a convenient criterion for characterizing the nonsingularity of the associated linear transformation, since d et ( A ) = F ( u 1, u 2,…, u n) = 0 if and only if the set of vectors u i ar e linearly dependent. There are many other useful and elegant properties of det erminants, most of which will be found in any classic book on linear algebra. Thus, det ( AB ) = det ( A ) det ( B ), and det ( A ) = det ( A') ,where A' is the transpose of A , obtained by the formula A' =( a ji ), thereby rotating the array about the main diagonal. If a square matrix is triangular, meaning th at all its entries above the main diagonal are 0,then det ( A ) turns out to be exactly the product of the diagonal entries.Another useful concept is that of eigenvalue. A scalar is said to be an eigenva lue for a transformation T if there is a nonzero vector υwith T (υ) λυ. It is then clear that the eigenvalues will be those numbers λ∈ K such that T -λI is a singular transformation. Any vector in the null space of T -λI is called an eigenvector of T associated with eigenvalue λ, and their span the eigenspace, E λ. It is invariant under the action of T , meaning that T carrie s Eλinto itself. The eigenvalues of T are then exactly the set of roots of the polynomial p(λ) =det ( T -λI ).If A is a matrix representing T ,then one h as p (λ) det ( A -λI ), which permits one to find the eigenvalues of T easil y if the dimension of V is not too large, or if the matrix A is simple enough. The eigenvalues and eigenspaces of T provide a means by which the nature and structure of the linear transformation T can be examined in detail.Vocabularylinear algebra 线性代数non-singular 非奇异field 域isomorphism 同构vector 向量isomorphic 同构scalar 纯量,无向量 matrix 矩阵(单数)vector space 向量空间matrices 矩阵(多数)span 生成,长成determinant 行列式independence 无关(性),独立(性) array 阵列dependence 有关(性) diagonal 对角线linear combination 线性组合 triangular 三角形的basis 基(单数) entry 表值,元素basis 基(多数) eigenvalue 特征值,本征值dimension 维eigenvector 特征向量linear transformation 线性变换 invariant 不变,不变量null space 零空间 row 行rank 秩 column 列singular 奇异 system of equations 方程组homogeneous 齐次Notes1. If U = { u 1, u 2,…}is a collection of points in a linearspace V , then the (linear) span of the set U is the set of all points of the form ∑c i u i , w where c i ∈K ,and all but a finite number of scalars c I are 0.意思是:如果U = { u 1, u 2,…}是线性空间V 的点集,那么集 U 的(线性)生成是所有形如∑c i u i的点集,这里c i ∈ K ,且除了有限个c i外均为0.2. A finite set { u 1, u 2,…, u k}is said to be linearly independent if the only way to write 0 = ∑c i u I is by choosing all the c i= 0.这一句可以用更典型的句子表达如下: A finite set { u 1, u 2,…, u k} is said to be linearly independen t in V if ∑c i u i is by choosing all the c i= 0.这里independent 是形容词,故用linearly修饰它. 试比较F(x) is a continuous periodic function.这里periodi c 是形容词但它前面的词却用continuous 而不用continuously,这是因为continuous 这个词不是修饰periodi c而是修饰作为整体的名词periodic function.3. Then these define isomorphisms of V onto K n and W onto K M respectively, and these in turn inducea linear transformation A between these.这里第一个these代表前句的两个基(basis);第二个these代表isomorphisms;第三个these代表什么留给读者自己分析.4. The least satisfactory aspect of linear algebra is still the theory of determinants-意思是:线性代数最令人不满意的方面仍是有关行列式的理论.least satisfactory 意思是:最令人不满意.5. If a square matrix is triangular, meaning that all its entries above the main diagonal are 0,then det ( A ) turns out to be exactly the product of the diagonal entries.意思是:如果方阵是三角形的,即所有在主对角线上方的元素均为零,那末det( A ) 刚好就是对角线元素的乘积.这里meaning that 可用that is to say 代替,turns out to be解为”结果是”.ExerciseI. Answer the following questions:1. How can we define the linear independence of an infinite set?2. Let T be a linear transformation (T: V →W ) whose associated matrix is A.Give a criterion for the non-singularity of the transformation T.3. Where is the entry a45of a m -by- n matrix( m>4; n>5) located ?4. Let A , B be two rectangular matrices.Under what condition is the product matrix well-defined ?II.Translate the following two examples and their proofs into Chinese:1.Example1. Let u k= t k ,k=0,1,2,... and t real. Show that the set ｛u 0,u1,u2,…｝is independent.Proof: By the definition of independence of an infinite set, it suffices to show that for each n ,the n+ 1 polynomials u0,u1,...,u n are independent.A relation of the form ∑n k=0c k u k=0 means ∑n k=0c k t k=0 for all t.When t=0,this gives c0=0.Differentiating both sides of ∑n k=0c k t k=0 and setting t=0,we fi nd that c1=0.Repeating the process,we find that each cocfficient is zero2. Example 2. Let V be afinite dimensional linear space, Then every finite basis for V has the same nu mber of elements.Proof: Let S and T be two finite bases for V. Suppose S consists of k elemnts and T consists of m e lements.Since S is independent and spans V ,every set of k+1 elements in V is dependent.Therefore eve ry set of more than k elements in V is dependent. Since T is an independent set , we must have m<k. The same argument with S and T interchanged shows that k<m. Hence k=m.III.Translate the following sentences into English:1.设 A 是一矩阵。

《数学专业英语》课件

PPT courseware for Mathematics Professional Englis
Introduction to Mathematics Professional EnglishMathematics Professional English VocabularyGrammar and Expression in Mathematical English
01
Definition and Importance
Course content: covering basic mathematical vocabulary, mathematical formulas and symbols, academic paper reading and writing, academic speeches and communication, mathematical literature translation, and other aspects.
Course objectives and content
Course objective: To cultivate students' mastery of basic vocabulary, grammar, and expression in mathematics related English, and to improve their English reading, writing, communication, and translation abilities in the field of mathematics.
English interface of software and tools
Learn how to read and understand English documentation for mathematical software and tools, master the professional terms and expressions in the documentation, and lay a foundation for in-depth learning and application.

数学专业英语词汇

1. 基本数学概念arithmetic mean 算术平均值weighted average 加权平均值geometric mean 几何平均数exponent 指数，幂base 乘幂的底数,底边cube 立方数，立方体square root 平方根cube root 立方根common logarithm 常用对数digit 数字constant 常数variable 变量inverse function 反函数 complementary function 余函数linear 一次的，线性的factorization 因式分解absolute value 绝对值，e.g.｜-32｜=32 round off 四舍五入2 有关数论natural number 自然数positive number 正数negative number 负数odd integer, odd number 奇数even integer, even number 偶数integer, whole number 整数positive whole number 正整数negative whole number 负整数 consecutive number 连续整数real number, rational number 实数,有理数 irrational（number）无理数inverse 倒数composite number 合数 e.g. 4,6,8,9,10,12,14,15……prime number 质数 e.g. 2,3,5,7,11,13,15……注意：所有的质数(2除外)都是奇数，但奇数不一定是质数reciprocal 倒数common divisor 公约数multiple 倍数(least)common multiple (最小)公倍数 (prime) factor (质)因子common factor 公因子ordinary scale, decimal scale 十进制 nonnegative 非负的tens 十位units 个位mode 众数median 中数common ratio 公比7. 数列arithmetic progression(sequence) 等差数列geometric progression(sequence) 等比数列8. 其它approximate 近似(anti)clockwise (逆) 顺时针方向 cardinal 基数ordinal 序数direct proportion 正比distinct 不同的estimation 估计，近似parentheses 括号proportion 比例permutation 排列combination 组合table 表格trigonometric function 三角函数unit 单位,位几何部分1. 所有的角alternate angle 内错角corresponding angle 同位角vertical angle 对顶角central angle 圆心角interior angle 内角exterior angle 外角supplementary angles 补角 complementary angle 余角adjacent angle 邻角acute angle 锐角obtuse angle 钝角right angle 直角round angle 周角straight angle 平角included angle 夹角2. 所有的三角形equilateral triangle 等边三角形 scalene triangle 不等边三角形 isosceles triangle 等腰三角形right triangle 直角三角形oblique 斜三角形inscribed triangle 内接三角形3. 有关收敛的平面图形，除三角形外semicircle 半圆concentric circles 同心圆 quadrilateral 四边形pentagon 五边形hexagon 六边形heptagon 七边形octagon 八边形nonagon 九边形decagon 十边形polygon 多边形parallelogram 平行四边形equilateral 等边形plane 平面square 正方形，平方rectangle 长方形regular polygon 正多边形rhombus 菱形trapezoid 梯形4. 其它平面图形arc 弧 line, straight line 直线line segment 线段parallel lines 平行线segment of a circle 弧形5. 有关立体图形cube 立方体，立方数rectangular solid 长方体regular solid/regular polyhedron 正多面体 circular cylinder 圆柱体cone 圆锥sphere 球体solid 立体的6. 有关图形上的附属物altitude 高depth 深度side 边长circumference, perimeter 周长radian 弧度surface area 表面积volume 体积arm 直角三角形的股cross section 横截面center of a circle 圆心chord 弦radius 半径angle bisector 角平分线diagonal 对角线diameter 直径edge 棱face of a solid 立体的面hypotenuse 斜边included side 夹边leg 三角形的直角边median of a triangle 三角形的中线base 底边，底数（e.g. 2的5次方，2就是底数）opposite 直角三角形中的对边midpoint 中点endpoint 端点vertex (复数形式vertices)顶点tangent 切线的transversal 截线intercept 截距7. 有关坐标coordinate system 坐标系 rectangular coordinate 直角坐标系 origin 原点abscissa 横坐标ordinate 纵坐标number line 数轴quadrant 象限slope 斜率complex plane 复平面8. 其它plane geometry 平面几何 trigonometry 三角学bisect 平分circumscribe 外切inscribe 内切intersect 相交perpendicular 垂直 pythagorean theorem 勾股定理 congruent 全等的multilateral 多边的其它1. 单位类cent 美分penny 一美分硬币nickel 5美分硬币dime 一角硬币dozen 打（12个）score 廿(20个)Centigrade 摄氏Fahrenheit 华氏quart 夸脱gallon 加仑(1 gallon = 4 quart) yard 码 meter 米micron 微米inch 英寸foot 英尺minute 分(角度的度量单位，60分=1度) square measure 平方单位制cubic meter 立方米pint 品脱(干量或液量的单位)2. 有关文字叙述题，主要是有关商业intercalary year(leap year) 闰年(366天) common year 平年(365天) depreciation 折旧down payment 直接付款discount 打折margin 利润profit 利润interest 利息simple interest 单利compounded interest 复利dividend 红利decrease to 减少到decrease by 减少了increase to 增加到increase by 增加了denote 表示list price 标价markup 涨价per capita 每人ratio 比率retail price 零售价tie 打。

数学专业英语

数学专业英语第二章精读课文-- 入门必修2.1 数学方程与比例（Mathematics，Equation and Ratio）一、词汇及短语：1. Cha nge the terms about变形2. full of ：有许多的充满的例The StreetS are full of people as on a holiday像假日一样，街上行人川流不息）3. in groups of ten??4. match SOmething against sb. “匹配”例Long ago ,when people had to Count many things ,they matChed them against their fingers. 古时候，当人们必须数东西时，在那些东西和自己的手指之间配对。

5. grow out of 源于由…引起例Many close friendships grew out of common acquaintance6. arrive at 得出（到达抵达达到达成）例We both arrived at the Same COnclusion我们俩个得出了相同的结论）7. stand for “表示，代表”8. in turn “反过来，依次”9. bring about 发生导致造成10. arise out of 引起起源于11. express by “用…表示”12. occur 发生，产生13. come from 来源于，起源于14. resulting method 推论法15. be equal to 等于的相等的例TWiCe two is equal to four（2 乘以 2 等于4）16. no matter 无论不管17. mathematical analysis 数学分析18. differential equation 微分方程19. higher mathematics 高等数学higher algebra 高等代数20. equation of condition 条件等式二句型及典型翻译1. For a long period of the history of mathematics, the centric place of mathematicalmethods was occupied by the logical deductions “在数学史的很长的时期内，是逻辑推理一直占据数学方法的中心地位”2. An equation is a statement of the equality between two equal numbers or numbersymbols.equation ：“方程”“等式” 等式是关于两个数或数的符号相等的一种陈述3. In such an equation either the two members are alike, or become alike onperformance of the indicated operation. 这种等式的两端要么一样，要么经过执行指定的运算后变成一样。

数学专业英语2-6

The word “function” was introduced into mathematics by Leibniz, who used the term primarily to refer to certain kinds of mathematical formulas. “函数”这个词是由莱布尼茨引入到数学中的，他主要使用这个术语来指代某种数学公式。 It was later realized that Leibniz’s idea of function was much too limited in its scope, and the meaning of the word has since undergone many stages of generalization. 后来人们才认识到，莱布尼茨的函数思想适用的范围太过局限了，这个术语的含义从那时起已经过了多次
These are merely devices for describing special relations in a quantitative fashion. Mathematicians refer to certain types of these relations as functions. 这些只是以定量的方式描述特定关系的方法。数学家将这些关系中的某些类型视作函数。 In this section, we give an informal description of the function concept. A formal definition is given in Section 3. 在本节中，我们给出一个非正式的描述函数的概念。在meaning of function is essentially this: Given two sets, say X and Y, a function is a correspondence which associates with each element of X one and one only element of Y.

《数学专业英语》课件

1 基础三角函数及其性质 3 正弦定理和余弦定理
2 三角恒等式和方程 4 三角学在几何和物理中的应用
IV. Calculus
1 极限和连续性 4 定积分及其性质
2 导数及其性质
3 导数在优化和相关速
率中的应用
5 定积分在面积和体积计算中的应用
V. Linear Algebra
1 向量和向量运算 3 线性方程组
《数学专业英语》PPT课件
探索数学专业英语的精髓，为您呈现一场精彩的数学之旅。
I. Introduction
- 数学的定义 - 数学在现代社会中的重要性 - 课程目标
II. Algebra
1 基础代数表达式和方程 3 多项式和因式分解
2 根式和指数 4 二次方程和函数
III. Trigonom etry
2 矩阵及其运算 4 特征值和特征向量
VI. Probability and Statistics
1 概率的基本概念 3 数据的统计度量
2 离散和连续概率分布 4 假设检验和置信区间
VII. Conclusion
1 课程内容回顾
2 数学在不同领域的未 3 继

数学专业英语

2 . 掌握概率论基本的表示方法。
11-A Predicates
Statements involving variables, such as “x>3”, “x+y=3”, “x+y=z” are often found in mathematical assertion and in computer programs.
Two types of quantification will be discussed here, namely, universal quantification and existential quantification . 这里讨论两种量词化方法，也就是全称量词化和存在量词化。
※Many mathematical statements assert that a property is true for all values of a variable in a particular domain, called the universe of discourse.
Assume we have a probability space (S,B,P) associated with an experiment. Let A be an event, and suppose the experiment is performed and that its outcome is x. (In other words, let x be a point of S.) 假设有一个对应于某一个试验的概率空间(S,B,P) 。A 是一个事件，假设试验已经完成，结果是x(换句话说， x是S中的一个点)。 This outcome x may or may not belong to the set A. If it does, we say that the event A has occurred. 结果x可能属于集合A，也可能不属于A。如果属于，则称事件A发生。

数学专业英语概述

林智 (浙大数学)
数学专业英语概述
2018年12月28日
10 / 39
常见错误分析
论文题目应准确、简洁地反映研究对象，既不过于空泛（使用像“On the study of...”或“On the existence of ...”这样的标题），也不过于烦琐（尽量不超过10个单词）文题通常是一个名词性短语，不是一个完整的句子。动词一般以“ing”形式出现。有时冠词可以省略偶尔有以疑问句为题目的论文，但不应以陈述句为题为了方便二次检索，避免使用复杂的数学符号和式子
但应全文
林智 (浙大数学)
数学专业英语概述
2018年12月28日
23 / 39
常见错误分析
动词：用法最复杂实例中错误较多的是单复数和时态数学论文中的动词多数情形用一般现在时即可，尤其是在证明过程中即使在反证法中，虚拟语态按理要用过去时，但学术界普遍都用现在时，没有人会提出异议或责难。
林智 (浙大数学)
数学专业英语概述
2018年12月28日
9 / 39
常见错误分析
评论前人工作时，要尊重引文作者叙述前人欠缺以强调自己创新时，应慎重并留有余地不要用“至今无人涉足这一方向”之类的语言，而应说“To the authors’ knowledge, there is little information in literature about ...”；不要说某人的结果是错误的，而应该说“They did not give a complete proof of ...”或“However, there is a gap in the proof of ...”
林智 (浙大数学)
数学专业英语概述

数学专业英语

数学专业英语数学，作为一门古老而深邃的学科，其影响力贯穿了人类文明的发展历程。

从古代的算术谜题到现代的复杂理论，数学始终在不断演进和拓展。

而随着全球化的推进以及学术交流的日益频繁，数学专业英语成为了数学领域中不可或缺的工具。

在数学的世界里，精准和清晰的表达至关重要。

数学专业英语正是为了实现这种精准性和清晰性而存在的。

它不仅仅是将数学概念翻译成英语，更是要遵循特定的语法规则、词汇用法和表达习惯，以确保信息的准确传递。

数学专业英语中的词汇具有很强的专业性。

例如，“function”（函数）、“derivative”（导数）、“integral”（积分）等，这些词汇在数学语境中有着特定且明确的含义。

与日常英语中的词汇相比，它们的定义更为精确和狭窄。

掌握这些专业词汇是理解和表达数学思想的基础。

数学专业英语的语法结构也有其特点。

为了准确地描述数学关系和逻辑，句子结构通常较为复杂，常常使用从句和被动语态。

例如，“The value which is obtained by solving this equation is cr ucial for our further analysis”（通过求解这个方程得到的值对于我们的进一步分析至关重要。

）这种复杂的语法结构有助于清晰地阐述数学推理过程。

数学专业英语在学术研究和交流中发挥着关键作用。

科研人员需要阅读国际上最新的数学研究成果，这就要求他们具备良好的数学专业英语阅读能力。

在撰写学术论文时，他们也必须用准确的数学专业英语来表达自己的研究思路和成果，以确保能够被国际同行理解和认可。

对于数学专业的学生来说，学好数学专业英语是提升自身能力和竞争力的重要途径。

在课程学习中，他们会接触到大量的英文教材和文献。

如果不能熟练掌握数学专业英语，就很难理解和掌握这些知识。

而且，在未来的深造和职业发展中，无论是参加国际学术会议还是与国外学者合作研究，都需要运用数学专业英语进行有效的沟通。