混凝土塑性损伤模型表格解读by自习菌
混凝土静动弹塑性损伤模型及在大坝分析中的应用共3篇

混凝土静动弹塑性损伤模型及在大坝分析中的应用共3篇混凝土静动弹塑性损伤模型及在大坝分析中的应用1混凝土材料是目前世界上应用最广泛的工程材料之一,常被用于建筑、桥梁、隧道、坝体、水利设施等重要工程中。
在大坝领域中,混凝土是大坝基础和主体结构的主要材料。
因此,混凝土的性质和性能对大坝的安全和稳定性具有至关重要的影响。
为了提高混凝土结构的耐久性、抗震性、抗风性能等,需要先了解混凝土材料的静动弹塑性损伤模型。
混凝土的静动弹塑性损伤模型是研究混凝土的物理性能、力学性能及受力特性的基础;它可以模拟混凝土在不同载荷状态下的变形和破坏过程,并分析混凝土受力后的力学特性。
一般而言,混凝土材料力学行为具有非线性、各向同性和单向松弛等特性。
因此,混凝土的静动弹塑性损伤模型应该考虑这些特性,包括弹性模量、泊松比、混凝土强度等参数,以及混凝土的动态强度变化等因素。
在大坝领域中,混凝土的静动弹塑性损伤模型应用广泛。
大坝结构承受巨大的水压力和地震力,混凝土在受力下会发生变形和破坏,甚至会引起坝体的塌陷和溃坝事故。
因此,为了保障大坝的安全和稳定性,需要在大坝建设过程中对混凝土的静动弹塑性损伤模型进行详细研究和应用。
大坝工程中,混凝土的静动弹塑性损伤模型可以用于模拟混凝土材料受力后的变形和损伤,预测混凝土结构的破坏点、残余强度和疲劳寿命,分析混凝土受水压力和地震力等外界因素的影响,为大坝的设计、施工和维护提供参考依据。
例如,在水电站大坝设计中,通常采用混凝土的静动弹塑性损伤模型进行分析,以确定大坝的结构类型、材料特性、结构参数等,以及设计水库的水位、底板的厚度和坝体的高度等。
在大坝的施工过程中,混凝土的静动弹塑性损伤模型可以用于进行质量监测和预警,及时发现混凝土结构的变形和损伤情况,预测混凝土的受力状况和疲劳状态,以确保大坝的稳定和安全。
在大坝的维护和修复中,混凝土的静动弹塑性损伤模型可以使用,对大坝进行评估和维护,以延长大坝的使用寿命。
混凝土的一种标量损伤弹塑性本构模型

σ εe ij ij
=
5Ψ 5εeij
=
1-
d
ε C : 0
e
ij kl kl
(6)
第 2 期
邵长江 ,等 : 混凝土的一种标量损伤弹塑性本构模型
299
及耗散不等式 :
Ψ0 εij ,εipj
·
d
≥0
(7)
可见
,
·
ABAQUS混凝土损伤塑性模型参数验证

第38卷第8期建 筑 结 构2008年8月ABAQUS 混凝土损伤塑性模型参数验证张 劲1 王庆扬1,2 胡守营1 王传甲2(1中国石油大学 北京102249;2中国电子工程设计院深圳市电子院设计有限公司 深圳581031)[摘要] 为了统一ABAQUS 混凝土损伤塑性模型与规范提供的混凝土本构模型,在规范提供的混凝土本构关系的基础上引入损伤因子的概念,对混凝土损伤塑性模型本构关系参数的确定方法进行了研究。
用各等级混凝土本构关系参数模拟结果与规范曲线的对比,验证CDP 模型参数的正确性;用一混凝土剪力墙试验的模拟分析,验证本构关系参数用于结构分析情况下的可靠性。
两种验证结果证明,给出的CDP 模型参数确定方法是正确的,用该方法确定的参数进行结构模拟分析所得结果是可靠的,并指出了CDP 模型的不足。
[关键词] ABAQUS ;混凝土损伤塑性模型;剪力墙试验Parameters Verification of Concrete Damaged Plastic Model of ABAQUS Zhang Jin 1,Wang Qingyang 1,2,Hu Shouying 1,Wang Chuanjia2(1China Univ .of Petroleu m ,Beijing 102249,China ;2Shenzhen Electronics Design Inst .Co .,Ltd .,Shenzhen 518031,China )A bstract :To uniform the concrete damaged plastic model provided by ABAQUS and the concrete constitutive relatiouships provided by the code for concrete structure design ,the damaged factors was introduced into the constitutive relationship provided by criterion ,and then the method used to determine the parameters of CDP model was studied .To verify the correctness of the parameters of CDP model ,the method of contrastin g the results extracted from simulation and the criterion curves is used ;and to verify the reliability applied to structure s imulation ,the method of contrasting s imulation results and experimental results is chosen .It is approved that the determined method of CDP model parameters is correct and the simulation results of structures using the parameters determined by the method is reliable .The shortage of CDP model was ind icated .Keywords :ABAQUS ;concrete damaged plastic model ;s hear wall test作者简介:张劲(1963-),男,副教授。
ABAQUS_混凝土损伤塑性模型_损伤因子

ABAQUS_混凝⼟损伤塑性模型_损伤因⼦混凝⼟损伤因⼦的定义BY lizhenxian271 损伤因⼦的定义损伤理论最早是1958年Kachanov提出来⽤于研究⾦属徐变的。
所谓损伤,是指在各种加载条件下,材料内凝聚⼒的进展性减弱,并导致体积单元破坏的现象,是受载材料由于微缺陷(微裂纹和微孔洞)的产⽣和发展⽽引起的逐步劣化。
损伤⼀般被作为⼀种“劣化因素”⽽结合到弹性、塑性和粘塑性介质中去。
由于损伤的发展和材料结构的某种不可逆变化,因⽽不同的学者采⽤了不同的损伤定义。
⼀般来说,按使⽤的基准可将损伤分为:(1) 微观基准量1,空隙的数⽬、长度、⾯积、体积;2空隙的形状、排列、由取向所决定的有效⾯积。
(2) 宏观基准量1、弹性常数、屈服应⼒、拉伸强度、延伸率。
2、密度、电阻、超声波波速、声发射。
对于第⼀类基准量,不能直接与宏观⼒学量建⽴物性关系,所以⽤它来定义损伤变量的时候,需要对它做出⼀定的宏观尺度下的统计处理(如平均、求和等)。
对于第⼆类基准量,⼀般总是采⽤那些对损伤过程⽐较敏感,在实验室⾥易于测量的量,作为损伤变量的依据。
由于微裂纹和微孔洞的存在,微缺陷所导致的微应⼒集中以及缺陷的相互作⽤,有效承载⾯积由A减⼩为A’。
如假定这些微裂纹和微孔洞在空间各个⽅向均匀分布,A’与法向⽆关,这时可定义各向同性损伤变量D为D= ( A- A’ )/ A事实上,微缺陷的取向、分布及演化与受载⽅向密切相关,因此材料损伤实际上是各向异性的。
为描述损伤的各向异性,可采⽤张量形式来定义。
损伤表征了材损伤是⼀个⾮负的因⼦,同时由于这⼀⼒学性能的不可逆性,必然有0dDdt≥ 2有效应⼒定义Cauchy 有效应⼒张量'σ''//(1)A A D σσσ==-⼀般情况下,存在于物体内的损伤(微裂纹、空洞)是有⽅向性的。
当损伤变量与受⼒⾯法向相关时,是为各向异性损伤;当损伤变量与法向⽆关时,为各向异性损伤。
混凝土弹塑性损伤本构模型研究

混凝土弹塑性损伤本构模型研究一、概述混凝土作为一种广泛应用于土木工程领域的重要建筑材料,其力学行为的研究对于工程结构的设计、施工和维护至关重要。
弹塑性损伤本构模型作为描述混凝土材料在复杂应力状态下力学行为的重要工具,近年来受到了广泛关注。
该模型能够综合考虑混凝土的弹性、塑性变形以及损伤演化等多个方面,为工程结构的非线性分析和损伤评估提供了有效的理论支持。
本文旨在深入研究混凝土弹塑性损伤本构模型的理论框架、数值实现及其在工程中的应用。
我们将对混凝土弹塑性损伤本构模型的基本理论进行梳理,包括模型的建立、参数的确定以及损伤演化方程的推导等方面。
通过数值模拟和试验验证相结合的方法,对模型的准确性和适用性进行评估。
我们将探讨该模型在土木工程结构非线性分析、损伤评估以及加固修复等方面的实际应用,为工程实践提供有益的参考和指导。
通过本文的研究,我们期望能够为混凝土弹塑性损伤本构模型的理论发展和工程应用提供新的思路和方法,推动土木工程领域相关技术的创新和发展。
1. 研究背景:介绍混凝土作为一种广泛应用的建筑材料,在土木工程中的重要性。
混凝土,作为土木工程领域中使用最广泛的建筑材料之一,其性能与行为对结构的整体安全性、经济性和耐久性具有至关重要的影响。
由于其独特的物理和力学性能,混凝土在桥梁、大坝、高层建筑、地下结构等各类土木工程设施中发挥着不可替代的作用。
随着工程技术的不断进步和建筑需求的日益增长,对混凝土材料性能的理解和应用要求也越来越高。
混凝土是一种非均质、多相复合材料,其力学行为表现出明显的弹塑性特性,并且在受力过程中可能产生损伤累积,进而影响其长期性能。
建立能够准确描述混凝土弹塑性损伤行为的本构模型,对于准确预测混凝土结构的受力性能、优化设计方案以及保障结构安全具有重要的理论和实际意义。
近年来,随着计算力学和材料科学的快速发展,对混凝土弹塑性损伤本构模型的研究已成为土木工程领域的研究热点之一。
通过对混凝土材料在复杂应力状态下的力学行为进行深入研究,建立更加精细和准确的本构模型,有助于提升对混凝土结构性能的认识,推动土木工程技术的进步与发展。
(仅供参考)Abaqus混凝土损伤塑性模型的参数标定

Abaqus 混凝土损伤塑性模型的参数标定1. 塑性参数(Plasticity )1) 剪胀角(Dilation Angle )= 30°2) 流动势偏移量(Eccentricity )3) 双轴受压与单轴受压极限强度比= 1.164)不变量应力比 = 0.667 5) 粘滞系数(Visosity Parameter) = 0.00052. 受压本构关系应力-Yield Stress :第一行应输入本构模型刚进入非弹性段非弹性应变为0时所对应的应力。
非弹性应变-Inelastic Strain (受拉时为开裂应变-Cracking Strain ):根据应力按混凝土本构模型得出对应的应变值,并通过, 和 ,得出非弹性应变。
3. 受压损伤因子(Damage Parameter )计算根据《Abaqus Analysis User's Manual (6.10)》 - 20.6.3 “Concrete damaged plasticity ”中公式:假设非弹性应变 in c ε中塑性应变 plc ε所占的比例为c β,通过转换可得损伤因子cd 的计算公式:() () 0011in c c in c c c c E E d βεσβε-=+-根据《ABAQUS 混凝土损伤塑性模型参数验证》规定,混凝土受压时c β的取值范围为0.35 ~ 0.7。
4. 受拉损伤因子(Damage Parameter )计算受拉损伤因子的计算与受压损伤因子的计算方法基本相同,只需将对应受压变量更换为受拉即可:() () 0011int t in t t t t E E d βεσβε-=+- 而根据参考文献混凝土受拉时t β的取值范围为0.5 ~ 0.95。
5. 损伤恢复因子受拉损伤恢复因子(Tension Recovery ):缺省值0t w =。
受压损伤恢复因子(Compression Recovery ):缺省值1c w =。
混凝土弹塑性损伤本构模型参数及其工程应用

( 1 . 中 国建 筑 股份 有 限 公 司 技 术 中心 , 北京 1 0 1 3 2 0 ; 2 . 同济 大 学 结 构 工 程 与 防 灾 研 究 所 , 上海 2 0 0 0 9 2 )
摘
要 :为 提 高 弹 塑 性 损 伤 本 构 模 型 的工 程 实用 性 , 研 究 各 参 数 取 值 对 模 型损 伤 发 展 、 塑 性 发 展 及 材 料 应 力 应 变 关
Ab s t r a c t :The v a r i a bl e s o f e l a s t i c p l a s t i c d a ma ge mo de l wa s s t ud i e d i n o r d e r t o i mpr o ve t he mod e l ’ S pr a c t i — c a bi l i t y s pe c i f i c a l l y .The e f f e c t o f d i f f e r e nt va r i a bl e s v a l ue s o n mo de l da ma g e d e v e l o pme n t ,pl a s t i c d e v e l o p— me nt a n d ma t e r i a 1 s t r e s s - s t r a i n r e l a t i o ns wa s s t u di e d. Th e f u nc t i on r e l a t i on s hi p wa s f i t t e d by c o nn e c t i ng t he pa r a me t e r s wi t h c o nc r e t e ma t e r i a l c ommon i n di c a t or s,i n c l ud i ng e l a s t i c mod ul u s。u ni a x i a l c ompr e s s i v e
CDP模型使用说明(BYsusoo88)

近些年,似乎abaqus 混凝土损伤塑性模型在结构工程(钢结构方向、混凝土方向)研究生论文中的普及率非常非常高,都试图采用abaqus 来模拟钢材/混凝土材料的受力性能,但在数值计算的过程中混凝土的损伤塑性模型的参数设置成了过不去的瓶颈,我也是深有感触,特别是研二刚开始学abaqus 的时候,非常痛苦,问师兄们也是一知半解,下面我把书上和论文中及自己的一些理解简捷的将abaqus 一些关键的操作和理论,并做成文件方便大家使用。
损伤模型在使用中主要是下面的截面:材料行为主要是密度、弹性、混凝土损伤塑性三类。
密度其实个人认为并不重要应该对计算没有太影响,至于不输有没影响我还没试过。
弹性中杨氏模量E。
对后面的计算影响很大应该慎重选取,后面会将到的,这里先按下不表。
泊松比的取值我都是参考别人的论文或是清华大学一次研究生作业中建议选取0.2 或0.164 这个现在想想还确实有些不明朗,大家可以将其作为参变量改变试试对计算结果的影响到底有多大。
混凝土损伤塑性主要有混凝土压缩损伤和混凝土拉伸损伤:塑性膨胀角(剪胀角)对混凝土的受力表现非常明显,至少我的型钢混凝土受扭中是非常明显的,一般在刚开始试算时建议取30°等其他数值确定下来可以调整该值将其趋近于试验数据,有点造假的意思,不过没办法这些数据的取值本就没一个固定的取值。
偏心率、fbo/fc0、K 分别是偏心率/流动势偏移量、双轴抗压强度与单轴抗压极限强度之比、不变量应力比,具体的解释大家可以去参考a baqus 的说明书,这边就不一一介绍。
粘性参数的取值直接关系到模型计算的收敛性,一般都不设置成0,我个人建议取值0.0005 或者0.005 看模型收敛效果而定。
该参数的改变对计算结果影响不大,(我的模型至少是这c0 c c acdctd 样,其他模型还真没试过) 下面最为关键的是受压行为和受拉行为中两列数据取值:这部分的取值是直接来源于你的混凝土单轴应力-应变关系曲线,这部分数值决定你模型受 混凝土单轴受压应力-应变关系方程:令 x/ 0 ,y / 0,其中0 , 0 分别为曲线的峰值应力、应变当 x0.211 即0.4 f *y ( E / f * )x当 0.211x 1时yx (3 2)x 2(2)x 3a a a其中:2.4 0.0125 f *当 x1 时yx(x 1)2 x其中:0.157 f *0.7850.905y=cfc1y=a x+(3-2a )x 2+(a -2)x 3y=cf t10.4xy=d (x-1)2+xy=(E 0c/f c )xy=x t (x-1)1.7+x1x=cy1x=ty图 1 混凝土受应力-应变关系图 2 混凝土受压拉应力-应变关系混凝土单轴受拉应力-应变关系方程: 当 x1时y 1.2x 0.2x 6当 x1 时yx( x 1)1.7 x其中: 0.312 f 2 ,f 为混凝土单轴抗拉强度。
混凝土塑性—损伤本构模型研究

混凝土塑性—损伤本构模型研究一、本文概述Overview of this article混凝土作为一种广泛应用的建筑材料,其力学性能和损伤行为的研究一直是土木工程领域的重要课题。
本文旨在深入研究和探讨混凝土塑性-损伤本构模型,该模型能够更准确地描述混凝土在复杂应力状态下的力学响应和损伤演化过程。
通过对混凝土塑性-损伤本构模型的研究,不仅有助于我们更好地理解混凝土的力学特性,还能为混凝土结构的设计、分析和优化提供理论基础和技术支持。
As a widely used building material, the study of mechanical properties and damage behavior of concrete has always been an important topic in the field of civil engineering. This article aims to conduct in-depth research and exploration on the plastic damage constitutive model of concrete, which can more accurately describe the mechanical response and damage evolution process of concrete under complex stress states. The study of the plastic damage constitutive model of concrete not only helps us better understand the mechanical properties ofconcrete, but also provides theoretical basis and technical support for the design, analysis, and optimization of concrete structures.本文首先介绍了混凝土塑性-损伤本构模型的基本概念和理论框架,包括塑性理论、损伤力学以及混凝土材料的特殊性质。
ABAQUS混凝土塑性损伤模型

4.5.2 混凝土和其它準脆性材料的塑性損傷模型這部分介紹的是ABAQUS提供分析混凝土和其它準脆性材料的混凝土塑性損傷模型。
ABAQUS 材料庫中也包括分析混凝的其它模型如基于彌散裂紋方法的土本構模型。
他們分別是在ABAQUS/Standard “An inelastic constitutive model for concrete,” Section 4.5.1, 中的彌散裂紋模型和在ABAQUS/Explicit, “A cracking model for concrete and other brittle materials,” Section 4.5.3中的脆性開裂模型。
混凝土塑性損傷模型主要是用來為分析混凝土結構在循環和動力荷載作用下的提供一個普遍分析模型。
該模型也適用于其它準脆性材料如巖石、砂漿和陶瓷的分析;本節將以混凝土的力學行為來演示本模型的一些特點。
在較低的圍壓下混凝土表現出脆性性質,主要的失效機制是拉力作用下的開裂失效和壓力作用下的壓碎。
當圍壓足夠大能夠阻止裂紋開裂時脆性就不太明顯了。
這種情況下混凝土失效主要表現為微孔洞結構的聚集和坍塌,從而導致混凝土的宏觀力學性質表現得像具有強化性質的延性材料那樣。
本節介紹的塑性損傷模型并不能有效模擬混凝土在高圍壓作用下的力學行為。
而只能模擬混凝土和其它脆性材料在與中等圍壓條件(圍壓通常小于單軸抗壓強度的四分之一或五分之一)下不可逆損傷有關的一些特性。
這些特性在宏觀上表現如下:單拉和單壓強度不同,單壓強度是單拉強度的10倍甚至更多;受拉軟化,而受壓在軟化前存在強化;在循环荷载(压)下存在刚度恢复;率敏感性,尤其是強度隨應變率增加而有較大的提高。
概論混凝土非粘性塑性損傷模型的基本要點介紹如下:應變率分解對率無關的模型附加假定應變率是可以如下分解的:是總應變率,是應變率的彈性部分,是應變率的塑性部分。
應力應變關系應力應變關系為下列彈性標量損傷關系:其中是材料的初始(無損)剛度,是有損剛度,是剛度退化變量其值在0(無損)到1(完全失效)之間變化,與失效機制(開裂和壓碎)相關的損傷導致了彈性剛度的退化。
混凝土塑性损伤模型1学习资料

混凝土塑性损伤模型1混凝土和其它准脆性材料的塑性损伤模型这部分介绍的是ABAQUS提供分析混凝土和其它准脆性材料的混凝土塑性损伤模型。
ABAQUS 材料库中也包括分析混凝的其它模型如基于弥散裂纹方法的土本构模型。
他们分别是在ABAQUS/Standard “An inelastic constitutive model for concrete,” Section 4.5.1, 中的弥散裂纹模型和在ABAQUS/Explicit, “A cracking model for concrete and other brittle materials,” Section 4.5.3中的脆性开裂模型。
混凝土塑性损伤模型主要是用来为分析混凝土结构在循环和动力荷载作用下的提供一个普遍分析模型。
该模型也适用于其它准脆性材料如岩石、砂浆和陶瓷的分析;本节将以混凝土的力学行为来演示本模型的一些特点。
在较低的围压下混凝土表现出脆性性质,主要的失效机制是拉力作用下的开裂失效和压力作用下的压碎。
当围压足够大能够阻止裂纹开裂时脆性就不太明显了。
这种情况下混凝土失效主要表现为微孔洞结构的聚集和坍塌,从而导致混凝土的宏观力学性质表现得像具有强化性质的延性材料那样。
本节介绍的塑性损伤模型并不能有效模拟混凝土在高围压作用下的力学行为。
而只能模拟混凝土和其它脆性材料在与中等围压条件(围压通常小于单轴抗压强度的四分之一或五分之一)下不可逆损伤有关的一些特性。
这些特性在宏观上表现如下:•单拉和单压强度不同,单压强度是单拉强度的10倍甚至更多;•受拉软化,而受压在软化前存在强化;•在循环荷载(压)下存在刚度恢复;•率敏感性,尤其是强度随应变率增加而有较大的提高。
概论混凝土非粘性塑性损伤模型的基本要点介绍如下:应变率分解对率无关的模型附加假定应变率是可以如下分解的:是总应变率,是应变率的弹性部分,是应变率的塑性部分。
应力应变关系应力应变关系为下列弹性标量损伤关系:其中是材料的初始(无损)刚度,是有损刚度,是刚度退化变量其值在0(无损)到1(完全失效)之间变化,与失效机制(开裂和压碎)相关的损伤导致了弹性刚度的退化。
混凝土弹塑性损伤本构模型研究_基本公式

·15 ·
应问题 , 但仍然难以给出合理 、有效的混凝土多维本 构关系 。问题的关键在于难以确立理论上合理 、与试 验吻合较好的损伤准则及相应的损伤演化法则 。
按照不可逆热力学的基本原理 , 应该采用与损伤 变量功共轭的热力学广义力 ———损伤能释放率建立损 伤准则[4~7] 。然而 , 此类损伤本构模型在多维应力状 态下的分析结果均与试验数据存在相当的差距 。为吻 合试验结果 , 部分损伤本构模型[8~12] 不得不放弃上 述热力学基础 , 而采用依据经验给定损伤准则的方 法。
不同 ; 荷载反向后受拉裂缝闭合导致材料刚度全部或 部分恢复 ; (2) 峰值应力后存在明显的刚度退化和强 度软化 ; (3) 双轴受压应力状态时材料强度和延性明 显增大 ; 双轴拉压应力下受压强度降低[1] (即所谓的 拉压软化效应[2]) ; (4) 超过一定阀值后 , 完全卸载 后存在不可恢复变形等 。
采用损伤力学的基本观点研究混凝土本构关系 , 有助于正确理解与反映混凝土材料的上述非线性特 性 。研究表明[3] , 经典的单标量损伤本构模型很难准 确地描述单边效应和混凝土多维本构关系 。采用合理 的双标量损伤变量虽可以较为有效地解决上述单边效
第 38 卷 第 9 期
李 杰等·混凝土弹塑性损伤本构模型研究
基于上述事实 , 在不考虑高静水压力导致的应变 强化的前提下 , 混凝土材料的损伤和破坏主要源于两 种不同的微观物理机制 , 即受拉损伤和受剪损伤机 制 。并可以采用受拉损伤变量 d + 和受剪损伤变量 d 来描述上述两种基本机制对材料宏观力学性能的影
混凝土塑形损伤模型参数计算研究

混凝土塑形损伤模型参数计算研究下载提示:该文档是本店铺精心编制而成的,希望大家下载后,能够帮助大家解决实际问题。
文档下载后可定制修改,请根据实际需要进行调整和使用,谢谢!本店铺为大家提供各种类型的实用资料,如教育随笔、日记赏析、句子摘抄、古诗大全、经典美文、话题作文、工作总结、词语解析、文案摘录、其他资料等等,想了解不同资料格式和写法,敬请关注!Download tips: This document is carefully compiled by this editor. I hope that after you download it, it can help you solve practical problems. The document can be customized and modified after downloading, please adjust and use it according to actual needs, thank you! In addition, this shop provides you with various types of practical materials, such as educational essays, diary appreciation, sentence excerpts, ancient poems, classic articles, topic composition, work summary, word parsing, copy excerpts, other materials and so on, want to know different data formats and writing methods, please pay attention!混凝土塑形损伤模型参数计算研究1. 引言混凝土在工程结构中具有广泛的应用,其力学性能对结构的耐久性和安全性至关重要。
ABAQUS钢筋混凝土损饬塑性模型有限元分析

ABAQUS钢筋混凝土损饬塑性模型有限元分析发表时间:2009-10-12 刘劲松刘红军来源:万方数据钢筋混凝土材料,是一种非匀质的力学性能复杂的建筑材料。
随着计算机和有限元方法的发展,有限元法已经成为研究混凝土结构的一个重要的手段。
由于数值计算具有快速、代价低和易于实现等诸多优点,这种分析方法已经广泛用于实际工程中。
然而,要在有限元软件中尽可能准确地模拟混凝土这种材料,是不容易的,国内外学者提出了基于各种理论的混凝土本构模型。
但是迄今为止,还没有一种理论被公认为可以完全描述混凝土的本构关系。
ABAQUS是大型通用的有限元分析软件,其在非线性分析方面的巨大优势,获得了广大用户的认可,在结构分析领域的应用趋于广泛。
本文把规范建议的混凝土本构关系,应用到损伤塑性模型,对一悬臂梁进行了精细的有限元建模计算和探讨。
1 混凝土损伤塑性模型ABAQUS在钢筋混凝土分析上有很强的能力。
它提供了三种混凝土本构模型:混凝土损伤塑性模型,混凝土弥散裂缝模型和ABAQUS/Explicit中的混凝土开裂模型。
其中混凝土损伤塑性模型可以用于单向加载、循环加载以及动态加载等场合,它使用非关联多硬化塑性和各向同性损伤弹性相结合的方式描述了混凝土破碎过程中发生的不可恢复的损伤。
这一特性使得损伤塑性模型具有更好的收敛性。
2 模型材料的定义2.1 混凝土的单轴拉压应力-应变曲线本模型中选用的混凝土本构关系是《混凝土结构设计规范》所建议的曲线,其应力应变关系可由函数表达式定义。
2.2 钢筋的本构关系钢筋采用本构关系为强化的二折线模型,无刚度退化。
折线第一上升段的斜率,为钢筋本身的弹性模量,第二上升段为钢筋强化段,此时的斜率大致可取为第一段的1/100。
2.3 损伤的定义损伤是指在单调加载或重复加载下,材料性质所产生的一种劣化现象,损伤在宏观方面的表现就是(微)裂纹的产生。
材料的损伤状态,可以用损伤因子来描述。
根据前面确定的混凝土非弹性阶段的应力一应变关系。
混凝土或其他脆性材料的塑性破坏

1/7Damaged plasticity model for con Crete and other quasi-brittle materialsProducts:Abaqus/Standard Abaqus/ExplicitThis secti on describes the con Crete damaged plasticity model provided in Abaqus for the an alysis of con Crete and otherquasi-brittle materials. The material library in Abaqus also in eludes other constitutive models for concrete based on the smeared crack approach.These are the smeared crack model inAbaqus/Standard’described in “Aninelastic constitutive model for concrete,M Section 4.5.1, and the brittle cracking model in Abaqus/Explicit, described in u A cracking modelfor concrete and other brittle materials,M Section 4.5.3.stiffness recovery effects during cyclic loading; andrate sen sitivity, especially an in crease in the peak st re ngth with strain rate. The plastic-damage model in Abaqus is based on the models proposed by Lubliner et al.(1989)and by Lee and Fenves(1998).The model is described in the remainder of this section.An overview of the main ingredients of the model is given first’followed by a more detailed discussion of the different aspects of the constitutive model.Overview2/7where is the total strain rate,is the elastic part of the strain rate, and is the plastic part of the strain rate.Stress-strain relationsThe stress-strain relations are go ver ned by scalar damaged elasticity: where is the initial (undamaged)elastic stiffness of the material;is the degraded elastic stiffness; and d is the scalar stiffness degradation variable, which can take values in the range from zero (undamaged material) to one (fully damaged material). Damage associated with the failure mecha nisms of the con Crete (cracki ng andcrushing) therefore results in a reduction in the elastic stiffness. Withi n the con text of the scalar-damage theory, the stiffness degradation is isotropic and characterized by a single degradationvariable,d.Following the usual notions of continuum damage mechanics, the effective stress is defined asThe Cauchy stress is related to the effective stress through the scalar degradation relation:For any given cross-section of the material,the factor represents the ratio of the effective load・carrying area (i.e., the overall area minus the damaged area) to the overall section area. In the absenee of damage…the effective stress is equivale nt to the Cauchystress, .When damage occurs, however, the effective stress is more representative than the Cauchy stress because it is the effective stress area that is resisting the external loads. It is, therefore, convenient to formulate the plasticity problem in terms of the effective stress.As discussed later,the evolution of the degradation variable is go ver ned by a set of harde ning variables,, and the effective stress; that is, .Hardening variablesas described later in this section.Microcracking and crushing in the concrete are represented by increasing values of the hardening variables.These variables control the evolution of the yield surface and the degradation of the elastic stiffness.They are also intimately related to the dissipated fracture en ergy required to gen erate micro-cracks.Yield functionThe yield function, / represents a surface in effective stress space, whichdetermi nes the states of failure or damage. For the in viscidplastic-damage modelThe specific form of the yield function is described later in this section. Flow rulePlastic flow is governed by a flow potential G according to the flow rule: where is the normegative plastic multiplier. The plastic potential is defined in the effective stress space.The specific form of the flow pote ntial for the con Crete damaged plasticity model is discussed later in this secti on .The model usesnon associated plasticity,therefore requiri ng the soluti on of nonsymmetric equations.SummaryIn summary, the elastic-plastic response of the concrete damaged plasticity model is described in terms of the effective stress and the harde ning variables:where and F obey the KuhrvTucker conditions:The Cauchy stress is calculated in terms of the stiffness degradation variable,, and the effective stress asThe constitutive relations for the elastic-plastic response,Equation4.5.2- 1,aredecoupled from the stiffness degradation response,Equation4.5.2一2,which makes the model attractive for an effectiven umerical impleme ntation. The in viscid model summarized here can be extended easily to account for viscoplastic effects through the use of a viscoplastic regularization by permitting stresses to be outside the yield surface.Damage and stiffness degradationThe evolution equations of the hardening variables and are conveniently formulated by considering uniaxial loading conditions first and then extended to multiaxial conditions.Uniaxial conditions4/7It is assumed that the un iaxial stress-strai n curves can be con verted into stress versus plastic strain curves of the formUnder uniaxial loading conditions the effective plastic strain rates are given asAs show n in Figure 4.5.2 - 1, when the con Crete specimen is unloaded from any point on the strainsoftening branch of thestress-strain curves, the unloading response is observed to be weake ned:The effective uniaxial cohesion stresses determine the size of the yield (or failure) surface.Uniaxial cyclic conditionsThe con Crete damaged plasticity model assumes that the reduction of the elastic modulus is given in terms of a scalar degradation variable, d, as where is the initial (undamaged) modulus of the material.where and are functions of the stress state that are introduced to represent stiffness recovery effects associated with stress reversals. They are defined according to whereThe evolutio n equatio ns of the equivale nt plastic strai ns are also generalized to the uniaxial cyclic conditions asThe evolution equations for the hardening variables must be extended for the general multiaxial conditions. Based on Lee and Fenves5/7(1998) we assume that the equivale nt plastic strai n rates are evaluated according to the expressions where and are, respectively, the maximum and minimum eigenvalues of the plastic strain rate tensor andIf the eigenvalues of the plastic strain rate tensor () are ordered such that, the evolution equation for general multiaxial stress conditions can be expressed in the following matrix form: where andElastic stiffness degradationThe plastic-damage con Crete model assumes that the elastic stiffness degradation is isotropic and characterized by a single scalar variable, d:similar to the uniaxial cyclic case, only that and are now given in terms of the function as It can be easily verified that Equation4.5.2一lOfor the scalar degradatio n varia ble is con sistent with the uniaxial response.and •Yield conditionThe plastic-damage concrete model uses a yield condition based on the yield function proposed by Lubliner et al.(1989) and in corporates the modificatio ns proposed by Lee and Fenveswhere and are dimensionless material constants; is the effective hydrostatic pressure; is the Mises equivalent effective stress;6/7is the deviatoric part of the effective stress tensor; and is the algebraically maximum eigenvalue of. The function is given as Typical experimental values of the ratio for concrete are in the range from 1.10 to 1.16, yielding values of between 0.08 and 0.12 (Lublineretal., 1989).Let for any given value of the hydrostatic pressure with ; thenThe fact that is constant does not seem to be contradicted by experimental evidenee (Lubliner et al., 1989). The coefficient is, therefore, evaluated asA value of, which is typical for concrete, givesLet for any given value of the hydrostatic pressure with ; then Typical yield surfaces are shown in Figure 4.5.2 - 4 in the deviatoric plane and in Figure 4.5.2 - 5for plane-stress conditions.Figure 4.5.2 - 4 Yield surfaces in the deviatoric plane, corresponding to different values of.Figure 4.5.2 一5 Yield surface in plane stress. Flow ruleThe plastic-damage model assumes non associated pote ntial flow, The flow potential G chosen for this model is the Drucker-Prager hyperbolic functio n:where is the dilation angle measured in the p - q plane at high confing pressure;is the uniaxial tensile stress at failure; and is a parameter, referred to as theeccentricity, that defines the rate at which the function approaches the asymptote (the flow potential tends to a straight line as the eccentricity tends to zero). This flow potential, which is continuous and smooth, en sures that the flow directi on is defi ned uniquely.The function asymptotically approaches the linear Drucker-Prager flowpote ntial at high con fing pressure stress and in tersects the hydrostatic pressure axis at 90" .See “ Modelsfor granular or polymer behavior, ” Section 4.4.2,for further discussion of this pote ntial.7/7Because plastic flow is non associated, the use of the plastic-damage concrete model requires the solution of nonsymmetric equations. Viscoplastic regularizationHere is the viscosity parameter representing the relaxation time of the viscoplastic system and is the plastic strain evaluated in the inviscid backbone model. Similarly, a viscous stiffness degradation variable,, for the viscoplastic system is defined as where d is the degradation variable evaluated in the inviscid backbone model.The stress-strain relation of the viscoplastic model is given as Integration of the modelThe model is integrated using the backward Euler method generally used with the plasticity models in Abaqus.A material Jacobian consistent with this integration operator is used for the equilibrium iterati ons.。
混凝土材料的塑性参数
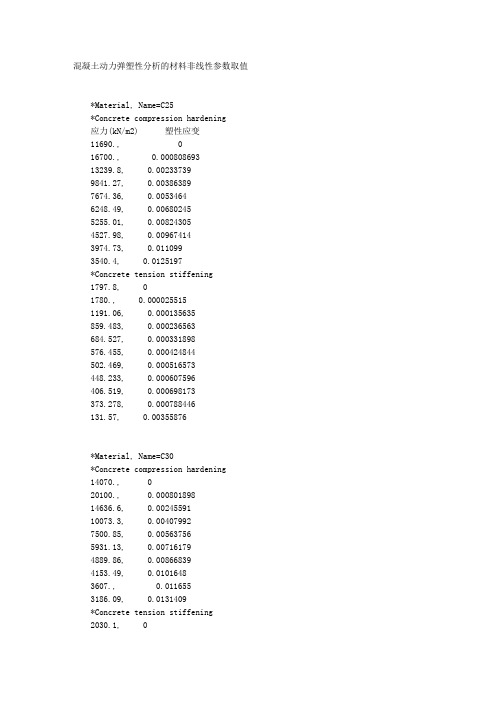
混凝土动力弹塑性分析的材料非线性参数取值*Material, Name=C25*Concrete compression hardening应力(kN/m2) 塑性应变11690., 016700., 0.00080869313239.8, 0.002337399841.27, 0.003863897674.36, 0.00534646248.49, 0.006802455255.01, 0.008243054527.98, 0.009674143974.73, 0.0110993540.4, 0.0125197*Concrete tension stiffening1797.8, 01780., 0.0000255151191.06, 0.000135635859.483, 0.000236563684.527, 0.000331898576.455, 0.000424844502.469, 0.000516573448.233, 0.000607596406.519, 0.000698173373.278, 0.000788446131.57, 0.00355876*Material, Name=C30*Concrete compression hardening14070., 020100., 0.00080189814636.6, 0.0024559110073.3, 0.004079927500.85, 0.005637565931.13, 0.007161794889.86, 0.008668394153.49, 0.01016483607., 0.0116553186.09, 0.0131409*Concrete tension stiffening2030.1, 01232.19, 0.00014944849.073, 0.000257466660.524, 0.000359008548.371, 0.000458002473.404, 0.000555757419.357, 0.000652815378.298, 0.00074944345.892, 0.000845777118.271, 0.00380631*Material, Name=C35*Concrete compression hardening 16380., 023400., 0.000789431 15814.6, 0.00256253 10267.4, 0.004270927408.77, 0.005893955749.74, 0.007478914682.74, 0.009045073943.69, 0.01060083403.29, 0.01215032991.69, 0.0136956*Concrete tension stiffening 2222., 02200., 0.00003014271253.05, 0.000160189834.315, 0.000273466638.442, 0.000379668524.938, 0.000483255450.278, 0.000585609397.041, 0.000687284356.924, 0.000788541325.457, 0.000889524109.188, 0.00399589*Material, Name=C40*Concrete compression hardening 18760., 026800., 0.000764814 16909.7, 0.00265856 10469.9, 0.004446145650.07, 0.00777334562.41, 0.00939623819.83, 0.01100853282.34, 0.01261442876.02, 0.0142164*Concrete tension stiffening 2413.9, 02390., 0.00003094221263.67, 0.000170079815.537, 0.000288349615.229, 0.000398993501.733, 0.000506966428.08, 0.000613712376.039, 0.000719794337.082, 0.000825474306.681, 0.00093089101.338, 0.00417611*Material, Name=C45*Concrete compression hardening 20720., 029600., 0.0007501517743.1, 0.00273782 10639.8, 0.004583597386.84, 0.006314435609.46, 0.008001224506.18, 0.009667883759.48, 0.01132393222.27, 0.01297372817.97, 0.0146195*Concrete tension stiffening 2535.1, 02510., 0.00003230441265.87, 0.000176673802.219, 0.000297743600.343, 0.000410999487.385, 0.0005216414.624, 0.000631002363.47, 0.000739759325.314, 0.000848128295.619, 0.00095624496.8983, 0.0042863*Material, Name=C50*Concrete compression hardening 22680., 032400., 0.000739885 18515.2, 0.00282136 10800.8, 0.004723987406.35, 0.006501395586.72, 0.008233144469.4, 0.009944553718.4, 0.01164533180.69, 0.01333992777.43, 0.0150306*Concrete tension stiffening 2666.4, 02640., 0.00003365911264.64, 0.000183705786.735, 0.000307738584.088, 0.000423793472.094, 0.00053722400.477, 0.000649477350.37, 0.00076111313.124, 0.00087237284.214, 0.00098338992.4472, 0.00440455*Material, Name=C55*Concrete compression hardening 24850., 035500., 0.00072745 19297., 0.0029113210959.8, 0.004873627429.12, 0.006700535569.63, 0.008480364439.05, 0.01023973683.89, 0.01198843145.52, 0.0137312743.03, 0.0154698*Concrete tension stiffening 2767.4, 02740., 0.00003522171261.67, 0.00018927774.457, 0.000315399571.752, 0.000433514460.697, 0.000549047390.039, 0.000663442340.767, 0.000777235304.231, 0.000890669275.923, 0.0010038789.2809, 0.00449368*Material, Name=C60*Concrete compression hardening 26950., 038500., 0.000700606 19978.3, 0.00298515 11087.9, 0.005002157444.03, 0.006873425552.82, 0.0086964412.21, 0.01049773654.2, 0.01228883115.66, 0.01407392714.07, 0.0158551*Concrete tension stiffening 2878.5, 02850., 0.00003563831256.67, 0.000194702760.756, 0.000323283558.434, 0.000443708448.564, 0.000561565379.016, 0.000678302330.681, 0.000794449294.926, 0.000910248267.272, 0.0010258286.0337, 0.00458981*Material, Name=C65*Concrete compression hardening 29050., 041500., 0.000671154 20635.5, 0.00305092 11227.6, 0.005116817477.58, 0.007027695554.81, 0.008888514402.81, 0.01072823640.4, 0.01255723100.27, 0.01438022698.31, 0.0161993*Concrete tension stiffening 2959.3, 02930., 0.00003624621252.05, 0.000198738750.74, 0.000328992548.949, 0.000451041440.021, 0.000570546371.305, 0.000688948323.655, 0.000806774288.463, 0.000924259261.278, 0.0010415283.8153, 0.00465851*Material, Name=C70*Concrete compression hardening 31150., 044500., 0.000641177 21274.9, 0.00311276 11377.9, 0.005224137526.44, 0.00717215571.79, 0.009068814407.16, 0.01094423639.02, 0.01280883096.11, 0.01466742692.74, 0.0165221*Concrete tension stiffening 3019.9, 02990., 0.0000369791248.1, 0.000201847743.23, 0.000333282541.959, 0.000456512433.772, 0.000577225365.689, 0.000696855318.553, 0.000815919283.779, 0.000934649256.941, 0.0010531682.2252, 0.00470937*Material, Name=C75*Concrete compression hardening 33180., 047400., 0.000619583 21842.2, 0.00318471 11504.1, 0.005343987565.26, 0.007332595583.9, 0.009269014408.93, 0.01118393636.25, 0.01308813091.21, 0.01498622686.84, 0.0168806*Concrete tension stiffening 3080.5, 03050., 0.00003771171243.9, 0.000204919735.834, 0.000337513535.156, 0.000461909427.722, 0.000583819360.269, 0.000704663313.639, 0.000824951279.275, 0.000944913252.774, 0.0010646680.7083, 0.00475965*Material, Name=C80*Concrete compression hardening 35140., 050200., 0.000601539 22358.2, 0.00325681 11618.5, 0.005462037601.82, 0.007490325596.63, 0.009465684412.34, 0.01141943635.46, 0.01336253088.38, 0.0152995 2683., 0.0172327*Concrete tension stiffening 3141.1, 03110., 0.00003844371239.38, 0.000207956728.476, 0.000341687528.471, 0.000467236421.811, 0.000590329354.989, 0.000712373308.862, 0.000833873274.904, 0.000955052248.735, 0.0010760379.2484, 0.00480935(6) 损伤系数:混凝土材料进入塑性状态伴随着刚度的降低, 如下图示(1):文献(3)Fig4. (a), (b) 给出了混凝土材料单轴拉压的滞回曲线. 该曲线已被实验和计算验证. 通过线性插值, 可以得到混凝土材料各塑性应变所对应的损伤系数如下: *Material, Name=C25*Concrete compression damage损伤系数(dc) 塑性应变0, 00.01, 0.0008086930.207199, 0.002337390.410702, 0.003863890.540458, 0.00534640.69718, 0.006802450.78611, 0.008243050.84114, 0.009674140.877465, 0.0110990.902661, 0.0125197*Concrete tension damage损伤系数(dt) 塑性应变0, 00.01, 0.0000255150.330864, 0.0001356350.517144, 0.0002365630.615434, 0.0003318980.747045, 0.0004248440.834016, 0.0005165730.888637, 0.0006075960.919064, 0.0006981730.937999, 0.0007884460.998225, 0.00355876*Material, Name=C30*Concrete compression damage0, 00.01, 0.0008018980.271809, 0.002455910.498841, 0.004079920.626823, 0.005637560.758068, 0.007161790.830984, 0.008668390.875447, 0.01016480.904483, 0.0116550.924462, 0.0131409*Concrete tension damage0, 00.01, 0.00002825630.386973, 0.000149440.577576, 0.000257466 0.671381, 0.000359008 0.78758, 0.0004580020.862336, 0.000555757 0.908476, 0.000652815 0.933922, 0.000749440.949638, 0.000845777 0.998607, 0.00380631*Material, Name=C35*Concrete compression damage 0, 00.01, 0.0007894310.324164, 0.002562530.561223, 0.004270920.683386, 0.005893950.796302, 0.007478910.858328, 0.009045070.89589, 0.01060080.92031, 0.01215030.937062, 0.0136956*Concrete tension damage0, 00.01, 0.00003014270.430433, 0.000160189 0.620766, 0.000273466 0.709799, 0.000379668 0.814503, 0.000483255 0.880713, 0.000585609 0.921137, 0.0006872840.943295, 0.000788541 0.956917, 0.000889524 0.998833, 0.00399589*Material, Name=C40*Concrete compression damage 0, 00.01, 0.0007648140.369042, 0.002658560.609331, 0.004446140.72467, 0.006130680.823245, 0.00777330.877128, 0.00939620.90969, 0.01100850.930844, 0.01261440.945352, 0.0142164*Concrete tension damage0, 00.01, 0.00003094220.471266, 0.000170079 0.658771, 0.000288349 0.742582, 0.000398993 0.836523, 0.000506966 0.895279, 0.000613712 0.930952, 0.000719794 0.95046, 0.0008254740.962428, 0.000930890.998995, 0.00417611*Material, Name=C45*Concrete compression damage 0, 00.01, 0.000750150.40057, 0.002737820.640546, 0.004583590.750445, 0.006314430.840063, 0.008001220.888862, 0.009667880.918306, 0.01132390.937422, 0.01297370.950532, 0.0146195*Concrete tension damage0, 00.01, 0.00003230440.495671, 0.000176673 0.680391, 0.000297743 0.760819, 0.000410999 0.849034, 0.00052160.903712, 0.000631002 0.936711, 0.000739759 0.954691, 0.000848128 0.965692, 0.000956244 0.999092, 0.0042863*Material, Name=C50*Concrete compression damage 0, 00.01, 0.0007398850.428544, 0.002821360.666642, 0.004723980.771409, 0.006501390.853722, 0.008233140.898389, 0.009944550.9253, 0.01164530.942763, 0.01333990.954738, 0.0150306*Concrete tension damage0, 00.01, 0.00003365910.52097, 0.0001837050.701994, 0.000307738 0.778754, 0.000423793 0.861159, 0.000537220.911801, 0.000649477 0.942194, 0.000761110.958699, 0.000872370.968775, 0.000983389 0.999181, 0.00440455*Material, Name=C55*Concrete compression damage 0, 00.01, 0.000727450.456423, 0.002911320.790729, 0.006700530.866219, 0.008480360.907065, 0.01023970.93165, 0.01198840.947601, 0.0137310.958541, 0.0154698*Concrete tension damage0, 00.01, 0.00003522170.539536, 0.000189270.717351, 0.000315399 0.791331, 0.000433514 0.869789, 0.000549047 0.91763, 0.0006634420.946178, 0.000777235 0.961623, 0.000890669 0.971027, 0.001003870.999246, 0.00449368*Material, Name=C60*Concrete compression damage 0, 00.01, 0.0007006060.481084, 0.002985150.712003, 0.005002150.806648, 0.006873420.876252, 0.0086960.913899, 0.01049770.936577, 0.01228880.951309, 0.01407390.961426, 0.0158551*Concrete tension damage0, 00.01, 0.00003563830.559063, 0.000194702 0.733068, 0.000323283 0.804058, 0.000443708 0.877995, 0.000561565 0.922909, 0.000678302 0.949666, 0.000794449 0.964133, 0.000910248 0.972937, 0.00102582*Material, Name=C65*Concrete compression damage 0, 00.01, 0.0006711540.502758, 0.003050920.729456, 0.005116810.819817, 0.007027690.884515, 0.008888510.919509, 0.01072820.940612, 0.01255720.95434, 0.01438020.96378, 0.0161993*Concrete tension damage0, 00.01, 0.00003624620.572681, 0.000198738 0.743775, 0.000328992 0.812645, 0.000451041 0.883611, 0.000570546 0.926569, 0.000688948 0.952106, 0.000806774 0.965898, 0.000924259 0.974284, 0.001041520.999336, 0.00465851*Material, Name=C70*Concrete compression damage 0, 00.01, 0.0006411770.521912, 0.003112760.744318, 0.005224130.830867, 0.00717210.891435, 0.009068810.924204, 0.01094420.943987, 0.01280880.956872, 0.01466740.965746, 0.0165221*Concrete tension damage0, 00.01, 0.0000369790.751428, 0.000333282 0.818743, 0.000456512 0.887672, 0.000577225 0.929255, 0.000696855 0.953916, 0.000815919 0.967215, 0.000934649 0.975292, 0.001053160.999364, 0.00470937*Material, Name=C75*Concrete compression damage 0, 00.01, 0.0006195830.539193, 0.003184710.757298, 0.005343980.840395, 0.007332590.897474, 0.009269010.928341, 0.01118390.946986, 0.01308810.95914, 0.01498620.967518, 0.0168806*Concrete tension damage0, 00.01, 0.00003771170.592163, 0.000204919 0.758743, 0.000337513 0.824539, 0.000461909 0.891519, 0.000583819 0.931794, 0.000704663 0.955624, 0.000824951 0.968456, 0.000944913 0.976241, 0.001064660.999391, 0.00475965*Material, Name=C80*Concrete compression damage 0, 00.01, 0.0006015390.554618, 0.003256810.768556, 0.005462030.848569, 0.007490320.931921, 0.0114194 0.94959, 0.0133625 0.961115, 0.0152995 0.969065, 0.0172327 *Concrete tension damage 0, 00.01, 0.0000384437 0.601485, 0.000207956 0.765763, 0.000341687 0.830074, 0.000467236 0.895181, 0.000590329 0.934206, 0.000712373 0.957244, 0.000833873 0.969631, 0.000955052 0.977139, 0.00107603 0.999416, 0.00480935。
混凝土结构耐久性外观损伤类型、调查方法及原因分析

混凝土结构耐久性外观损伤类型、调查方法及原因分析惠云玲(国家工业建筑诊断与改造工程技术研究中心 100088)郭 固(珠海经济特区房地产开发总公司 519000) 摘 要:论述了混凝土耐久性损伤类型,提出了混凝土结构外观损伤和环境条件的调查方法及原因分析,可为混凝土结构耐久性检测、评估提供一定的参考。
关键词:混凝土结构 耐久性 外观损伤INVEST IGAT I ON M ETHOD AND CAUSE ANALY SIS OF APPARENT DAM AGE OF D URAB I L IT Y FOR CONCRETE STRUCTURESH ui Yunling(N ati onal Engineering T echnique R esearch Center of Industrial Building D iagno sis&R ehabilitati on 100088)Guo Gu(Zhuhai S.E.Z.R eal E state D evelopm ent Co rp. 519000)Abstract:T he types of durability dam ages of concrete are described.T he m ethod fo r investigating apparent dam age and environm ental conditi ons of concrete structures and the m ethod of cause analysis are p ropo sed, w h ich can be used as a reference w hen detecting and esti m ating durability of concrete structures. Keywords:concrete structure durability apparent dam age1 混凝土结构耐久性损坏类型混凝土结构的耐久性体现了结构使用过程中抵抗各种破坏因素作用的能力。
- 1、下载文档前请自行甄别文档内容的完整性,平台不提供额外的编辑、内容补充、找答案等附加服务。
- 2、"仅部分预览"的文档,不可在线预览部分如存在完整性等问题,可反馈申请退款(可完整预览的文档不适用该条件!)。
- 3、如文档侵犯您的权益,请联系客服反馈,我们会尽快为您处理(人工客服工作时间:9:00-18:30)。
ABAQUS塑性损伤模型计算表格解读by自习菌(wx公众号)
受压本构:
fc,r:砼单轴抗压强度标准值,可根据需要取多种值,此处取fck轴心抗压强度标准值
fck:C30,20.1MPa;C35,23.4;C40,26.8;C45,29.6;C50,32.4;C55,35.5;C60,38.5
εcr:与单轴抗压强度fc,r相应的峰值压应变,规范附录公式
αc:单轴受压应力-应变曲线下降段的形状参数,规范附录公式
εcu:应力-应变曲线下降段0.5 fc,r对应的压应变
εcu/εcr:规范附录公式
可适当修正抗压强度代表值fcr,峰值压应变εcr,以及曲线形状参数αc,砼规C.2.4附录。
Ec:弹性模量,只是辅助计算的一个临时取值。
C30,3e4MPa;C40,3.25e4;C50,3.45e4
ρc:规范公式
n:规范公式
x:穷举数列,按规范公式与ε、εcr相关
dc:单轴受压损伤演化参数,以x=1为界限,规范为分段公式
ε:由x计算出,规范公式
σ:规范公式
σ修正:在应力-应变曲线上选定弹性阶段与弹塑性阶段的分界点,按Susoo88取0.4 fc,r,
或取1/3~1/2 fc,r,可见这也是一个可调整的值。
通过这个选定的点的应力应变,计算弹性阶段的斜率,即E0弹性模量,这个弹性模量就是所采用本构的弹性模量,用E0和ε再重新反算弹性阶段的σ,即得“σ修正”。
对于C30砼,fc,r 取fck=20.1MPa,0.4*20.1=8.04MPa,在表格中插入一行,定义一个ε值,使σ无限逼近8.04(此时尚需重新定义表格这一行x列公式,使之由ε列导出)。
根据这个应力应变值,求出E0,再由E0修正弹性阶段的应力值(即插入行之上的部分)。
【Susoo88:受压曲线与受拉曲线弹性临界点不一样,会产生两个弹性模量,需要在输入时选较大值,不然在后面导算等效塑性应变时会出错…】
σtrue,εtrue:之前得到的应力应变是“名义”应力应变,需要在此转换成真实应力应变。
表格中的红色部分为abaqus中的输入数据。
屈服应力,采用真实应力
非弹性应变,公式见表格及Susoo88,第一行要手动取0,否则软件报错
bc、塑性应变、损伤因子,公式见表格及Susoo88文章
最后一列的塑性应变检查,塑性应变值应随着非弹性应变的增加而增大,且不为负值,否则软件报错。
表格中Subopions是啥?
受拉本构:
按规范公式,受拉应力应变曲线在从0到ftr并非线性变化,Susoo88文章有误。
但表格中E0的计算却也认为是线性变化,与规范不一致?
是否也应仿照受压本构选取一个点指定为弹性阶段的临界点?
ρt通过Ec定义,为什么不直接用E0定义?
此处E0是否应按Susoo88所说跟受压时的E0取大值?
按照受拉时E0的计算方法,临界点即取为峰值点,故由此计算的弹性模量几乎恒小于受压时的E0。
据此,受拉本构在abaqus中只用输入峰值之后的下降段。
表格中的红色部分为abaqus中的输入数据。
开裂应变,定义方式同“非弹性应变”。
bt、塑性应变、损伤因子,公式见表格及Susoo88文章
最后一列的塑性应变检查,塑性应变值应随着非弹性应变的增加而增大,且不为负值,否则软件报错。
by自习菌。
